(精品)浙大生物统计样卷2010-2011 A答案
生物统计附试验设计a卷【考试试卷答案】

生物统计附试验设计a 卷【考试试卷答案】第 页,共1页1 生物统计附试验设计课程考试试卷(A )适用专业: 考试日期:试卷所需时间:120分钟 闭卷 试卷总分:100一、填空 (共3小题,每空1分,共8分)1.进行方差分析的基本前提是 、 、 。
2.常用的描述数据集中程度的统计量有 、 、 等。
3.根据研究目的确定的研究对象的全体称为 ,其中的一个研究单位称为 。
二、是非题(共3小题,每小题6分,共18分)以下命题均来自线性回归分析一章,请指出下列命题是否正确,并解释原因。
1.自变量X 、依变量Y 的单位改变的时候,一元线性回归方程保持不变。
2.如果能根据X 的数值推算Y ,则两者之间必然存在因果关系。
3.设中学生的身高Y (米)和年龄X (岁)的回归方程为Y=0.5+0.06X ,则初生婴儿的平均身高为0.5米。
三、简答题(共4小题,每小题6分,共24分)1.简述假设检验的步骤。
2.简述假设检验过程中,两种类型的错误的定义。
3.为什么不能用t-检验替代方差分析?4.什么是标准化回归系数?计算标准化回归系数有什么作用?四、实例分析题(共2小题,第1小题15分,第2小题20分,共35分)1.采用新疗法后,某种疾病的治愈人数与接受治疗人数之比(治愈率)较去年有所提高,经过假设检验,P<0.05。
以下结论哪些是正确的,哪些是错误的,并对错误的结论给出解释。
1)出现第Ⅰ类错误的可能性低于5%。
2)P 值越小,临床意义越大。
3)P<0.05说明治愈率的改善具有统计学意义,因此新疗法值得临床推广。
2.为研究赖氨酸对儿童生长发育的影响,拟在面包中加入赖氨酸对幼儿园的学生进行干预实验。
1)该试验如何设置对照?2)有哪些干扰因素需要控制?如何控制?五、试验分析题(共1题,每小题15分,共15分)1.为研究雌激素对子宫发育的影响,现有4窝不同品系未成年的大白鼠,每窝3只,随机分别注射不同剂量的雌激素,然后在相同条件下试验,并称得它们的子宫重量,见表1,试作方差分析。
生物统计期末考试试题

2010-2011(1)生物统计期末考试1、用两种电极测定同一水样的10个样品的pH值,结果如下表。
试问两种电极测定的结果有无差异?A电极 5.78 5.74 5.84 5.80 5.80 5.79 5.82 5.81 5.85 5.78 B电极 5.82 5.87 5.96 5.89 5.90 5.81 5.83 5.86 5.90 5.802、为了从3种不同饲料和3种不同温度中选择使鱼产量最高的水平组合,设计了两因素试验,每一水平组合重复4次,结果如下表,试进行方差分析。
用不同原料及不同温度的鱼产量(单位kg)原料温度BB1(30℃) B2(35℃) B3(40℃)A141 49 23 25 11 12 25 24 6 22 26 11A247 59 50 40 43 38 33 36 8 22 18 14A348 35 53 59 55 38 47 44 30 33 26 19 3、研究某种渔药的用量(x,kg/亩)和施用后在鱼体中的残留量(y,mg/kg)的关系,结果列于下表,试作直线回归分析。
x(kg/亩)0.5 1.0 1.5 2.0 2.5y(mg/kg)0.07 0.11 0.14 0.18 0.24、某渔场从10组亲鱼产生的后代中,每组抽出性别相同、体重接近的2条,将每组2条鱼随机地分配到两个饲料组,进行饲料对比试验,试验时间30天,增5、为了比较4种饲料(A)和鱼的3个品种(B),从每个品种随机抽取4尾鱼(共12尾)分别喂以4种不同饲料。
随机配置,分池饲养、位置随机排列。
100天后分别测出每尾鱼的日增重(g),数据如下,试检验饲料及品种间的差异显著性。
4种饲料3个品种鱼30日增重A 1A2A3A4B1505 545 590 530B2490 515 535 505B3445 515 510 4956、10尾鱼的饲料消耗(x)和增重(y)资料如下表(单位:g),试对增重与饲y 33 11 42 24 38 44 38 37 30 357 水体中NaCl含量对植物的生长有很大的影响,NaCl含量过高,将增加组织内无机盐的积累,抑制植物的生长。
生物统计考试题及答案

生物统计考试题及答案一、选择题(每题2分,共20分)1. 在生物统计中,下列哪项不是描述性统计的内容?A. 集中趋势的度量B. 离散程度的度量C. 概率分布D. 数据的收集和整理答案:C2. 以下哪个参数是衡量数据离散程度的?A. 平均数B. 中位数C. 方差D. 众数答案:C3. 假设检验中,P值的意义是什么?A. 拒绝原假设的概率B. 原假设为真时,观察到的统计量或更极端情况出现的概率C. 原假设为假时,观察到的统计量或更极端情况出现的概率D. 原假设为真时,观察到的统计量或更极端情况不出现的概率答案:B4. 以下哪种分布是描述二项分布的?A. 正态分布B. 泊松分布C. t分布D. F分布答案:A5. 在方差分析中,F值是如何计算的?A. 组间方差除以组内方差B. 组内方差除以组间方差C. 组间方差除以样本量D. 组内方差除以样本量答案:A6. 相关系数的取值范围是多少?A. -1到1B. -∞到∞C. 0到1D. 1到∞答案:A7. 以下哪种统计图适合展示分类数据的分布?A. 散点图B. 直方图C. 箱线图D. 饼图答案:D8. 以下哪种方法用于估计总体参数?A. 描述性统计B. 推断性统计C. 相关性分析D. 回归分析答案:B9. 在回归分析中,残差平方和(SSE)表示什么?A. 预测值与实际值之间的差异B. 实际值与平均值之间的差异C. 预测值与平均值之间的差异D. 预测值与预测值之间的差异答案:A10. 以下哪种检验用于比较两个独立样本的均值差异?A. t检验B. 方差分析C. 卡方检验D. 相关性检验答案:A二、填空题(每题2分,共20分)1. 在生物统计中,数据的类型通常分为______数据和______数据。
答案:定量,定性2. 正态分布的数学期望是______,标准差是______。
答案:μ,σ3. 假设检验中,如果P值小于显著性水平α,则我们______原假设。
答案:拒绝4. 方差分析中,组间方差与组内方差的比值称为______值。
生物统计试题总结及答案

生物统计试题总结及答案一、单项选择题1. 生物统计中,描述数据集中趋势的度量是()。
A. 方差B. 标准差C. 均值D. 极差答案:C2. 在统计学中,标准差是用来衡量()。
A. 数据的分布范围B. 数据的集中趋势C. 数据的离散程度D. 数据的偏态情况答案:C3. 以下哪个选项不是描述数据分布的统计量()。
A. 均值B. 中位数C. 众数D. 方差答案:A4. 假设检验中,P值小于显著性水平α时,我们通常会()。
A. 拒绝零假设B. 接受零假设C. 无法判断D. 重新收集数据答案:A5. 相关系数的取值范围是()。
A. -1到1之间B. 0到1之间C. -1到0之间D. 0到无穷大答案:A6. 在回归分析中,决定系数R²表示的是()。
A. 回归方程的斜率B. 解释变量对预测变量的解释程度C. 预测变量的方差D. 解释变量的方差答案:B7. 以下哪个选项是生物统计中常用的非参数检验()。
A. t检验C. 卡方检验D. 曼-惠特尼U检验答案:D8. 以下哪个选项是描述数据离散程度的统计量()。
A. 均值B. 中位数C. 众数D. 极差答案:D9. 在生物统计中,如果样本量足够大,根据中心极限定理,样本均值的分布将近似于()。
A. 正态分布B. 均匀分布D. 二项分布答案:A10. 以下哪个选项是描述数据偏态的统计量()。
A. 偏度B. 峰度C. 标准差D. 方差答案:A二、多项选择题1. 下列哪些是描述数据集中趋势的统计量()。
A. 均值B. 中位数C. 众数D. 方差答案:ABC2. 在假设检验中,以下哪些因素会影响P值的大小()。
A. 样本量B. 显著性水平αC. 效应大小D. 数据的分布答案:ACD3. 以下哪些是描述数据分布形状的统计量()。
A. 偏度B. 峰度C. 标准差D. 极差答案:AB4. 在回归分析中,以下哪些因素会影响R²的值()。
A. 解释变量的数量B. 解释变量与预测变量的相关性C. 样本量D. 预测变量的方差答案:ABC5. 以下哪些是生物统计中常用的参数检验()。
生物统计试题总结及答案

生物统计试题总结及答案一、选择题1. 下列哪项是生物统计学的主要研究内容?A. 生物数据的收集B. 生物数据的分析C. 生物数据的解释D. 以上都是答案:D2. 统计学中,总体是指:A. 研究对象的个体B. 研究对象的全体C. 研究对象的样本D. 研究对象的子集答案:B3. 描述数据集中趋势的统计量是:A. 平均数B. 中位数C. 众数D. 以上都是答案:D二、填空题1. 统计学中的________是指在一定条件下,可能发生也可能不发生的事件。
答案:随机事件2. 在生物统计学中,________是用来描述数据分布形态的统计量。
答案:偏度3. 回归分析中,________系数表示自变量对因变量的影响程度。
答案:回归三、简答题1. 简述生物统计学在生物科学研究中的应用。
答案:生物统计学在生物科学研究中的应用包括:实验设计、数据收集、数据分析、结果解释和科学决策等。
2. 描述一下生物统计学中的假设检验。
答案:假设检验是生物统计学中的一种方法,用于根据样本数据对总体参数进行推断。
它包括提出假设、选择适当的检验方法、计算检验统计量和做出决策等步骤。
四、计算题1. 已知某生物实验中,一组数据的平均数为10,标准差为2,求这组数据的变异系数。
答案:变异系数 = 标准差 / 平均数 = 2 / 10 = 0.22. 假设某生物实验中,两组数据的均值分别为5和7,标准差分别为1和1.5,求两组数据的均值差异的置信区间(置信度为95%)。
答案:首先需要计算两组数据的均值差异的标准误差,然后使用t分布表查找相应的t值,最后计算置信区间。
具体计算过程略。
五、论述题1. 论述生物统计学在现代生物技术发展中的重要性。
答案:生物统计学在现代生物技术发展中的重要性体现在:它提供了科学的数据收集和分析方法,帮助科研人员从大量数据中提取有价值的信息,从而推动了生物科学的进步。
此外,生物统计学还有助于提高实验设计的合理性和数据分析的准确性,减少实验误差,提高研究结果的可靠性。
生物统计答案A【考试试卷答案】

《生物统计附试验设计》试卷A 答案一、填空题1.DPS ,SAS ,SPSS ,R,等2.总体,个体二、简答题1.统计假设测验的步骤可总结如下:(1)提出无效假设H0和备择假设HA 。
(2)确定显著水平a ,此显著水平为犯第一类错误的概率。
(3)在H0为正确的假定下,根据统计数的抽样分布规律,算出实得差异由误差造成的概率;或划出否定区域。
(4)将算得的概率和a 相比较,或者将试验结果和否定区域相比较,从而作出接受或否定假设的推断。
2.1)效应可加性2)分布正态性3)方差同质性3.t 检验法适用于样本平均数与总体平均数及两样本平均数间的差异显著性检验,但在生产和科学研究中经常会遇到比较多个处理优劣的问题,即需进行多个平均数间的差异显著性检验。
这时,若仍采用t 检验法就不适宜了。
这是因为:1)检验过程烦琐2)无统一的试验误差,误差估计的精确性和检验的灵敏性低 对同一试验的多个处理进行比较时,应该有一个统一的试验误差的估计值。
若用t 检验法作两两比较,由于每次比较需计算一个21x x S -,故使得各次比较误差的估计不统一,同时没有充分利用资料所提供的信息而使误差估计的精确性降低,从而降低检验的灵敏性,在用t 检法进行检验时,由于估计误差的精确性低,误差自由度小,使检验的灵敏性降低,容易掩盖差异的显著性。
3)推断的可靠性低,检验的I 型错误率大 即使利用资料所提供的全部信息估计了试验误差,若用t 检验法进行多个处理平均数间的差异显著性检验,由于没有考虑相互比较的两个平均数的秩次问题,因而会增大犯I 型错误的概率,降低推断的可靠性。
由于上述原因,多个平均数的差异显著性检验不宜用t 检验,须采用方差分析法。
4.计算得到的回归方程称作标准化回归方程,相应的回归系数即为标准化回归系数。
标准化回归系数没有单位,可以用来比较各个自变量Xi对y的影响强度,通常在有统计学意义的前提下,标准化回归系数的绝对值越大,说明相应自变量对y的作用越大。
生物统计学试题集及答案

生物统计学试题集及答案一、单项选择题(每题2分,共20分)1. 生物统计学中,用于描述数据集中趋势的指标是()。
A. 方差B. 标准差C. 平均数D. 极差答案:C2. 下列哪个统计图最适合展示分类数据的分布情况?()A. 条形图B. 折线图C. 散点图D. 饼图答案:D3. 在生物统计中,用于比较两个独立样本均值差异的统计方法是()。
A. t检验B. 方差分析C. 卡方检验D. 相关性分析答案:A4. 相关系数的取值范围是()。
A. -1到1B. 0到1C. 0到正无穷D. 负无穷到正无穷答案:A5. 以下哪个选项不是描述数据离散程度的统计量?()A. 极差B. 方差C. 标准差D. 平均数答案:D6. 在进行回归分析时,用于衡量模型拟合优度的统计量是()。
A. R平方B. F统计量C. t统计量D. p值答案:A7. 卡方检验主要用于()。
A. 比较两个独立样本的均值B. 比较两个相关样本的均值C. 检验分类变量的独立性D. 检验回归模型的有效性答案:C8. 以下哪个选项是生物统计学中用于描述数据分布形态的统计量?()A. 峰度B. 偏度C. 标准差D. 方差答案:B9. 在生物统计学中,用于比较三个或以上样本均值差异的统计方法是()。
A. t检验B. 方差分析C. 卡方检验D. 相关性分析答案:B10. 以下哪个选项不是生物统计学中用于描述数据分布集中趋势的统计量?()A. 中位数B. 众数C. 四分位数D. 平均数答案:C二、多项选择题(每题3分,共15分)11. 在生物统计学中,以下哪些统计量可以用来描述数据的集中趋势?()A. 平均数B. 中位数C. 众数D. 方差答案:ABC12. 以下哪些统计图可以用于展示两个变量之间的关系?()A. 散点图B. 条形图C. 折线图D. 饼图答案:AC13. 在生物统计学中,以下哪些统计量可以用来描述数据的离散程度?()A. 极差B. 方差C. 标准差D. 峰度答案:ABC14. 以下哪些统计方法可以用来比较两个相关样本的均值差异?()A. t检验B. 配对t检验C. 方差分析D. 卡方检验答案:B15. 以下哪些统计量可以用来衡量回归模型的拟合优度?()A. R平方B. F统计量C. p值D. 标准差答案:AB三、判断题(每题2分,共10分)16. 生物统计学中的t检验只能用于比较两个样本的均值差异。
生物统计考试试卷及答案

1 / 11《生物统计附试验设计》复习题一、名词解释题1、样本与样本含量2、区间估计3、正态分布4、试验设计5、样本标准误6、Ⅱ类错误7、卡方的连续性矫正8、相关系数二、单项选择题(从每小题的备选答案中,选出正确答案,并将正确答案的番号填入题干的括号内)1、从一个总体中抽出一个样本,其观察值为23、24、25、26、27、28、29,则样本方差为( )。
A 、28/5B 、4C 、14/3D 、28 2、样本方差S 2=( )。
A 、)1()(2--∑n x x B 、n x x ∑-2)( C 、N x /)(2∑-μ D 、)1/()(2--∑n x μ3、一元线性相关与回归分析中,相关系数与回归系数的关系有r 2=( )。
A 、22xy yx b b B 、bxy byx . C 、xy yx b b D 、2b4、一元回归分析中,回归自由度为( )。
A 、n-1B 、n-2C 、n-3D 、1 5、若x ~N(10,4),P(x ≥12)等于( )。
A 、0.9545 B 、0.1587 C 、0.0938 D 、0.68276、某样本有n 个观察值,其乘积开n 次方根所得的值即为( )。
A 、算术平均数 B 、调和平均数 C 、几何平均数 D 、中位数7、显著性检验中,否定或接受无效假设的依据是( )。
A 、中心极限定理B 、小概率原理C 、方差分析原理D 、数学模型8、若x ~B (5,0.7),则P (x =0)等于( )。
A 、0B 、0.3500C 、0.3000D 、0.00243 9、下列关于平均数的叙述不正确的是( )。
2 / 11A 、平均数是资料的代表数B 、样本平均数服从或逼近正态分布C 、离均差的平方和为零D 、样本平均数是总体平均数的无偏估计值 10、t 检验中,若)(05.0df t t <,则表明样本实得差异由误差引起的概率( )。
A 、P >1%B 、1%<P <5%C 、P <1%D 、P >5% 11、显著性检验中,同时降低犯Ⅰ和Ⅱ型错误的可能性的根本办法是( )。
生物统计学试题集及答案

生物统计学试题集及答案生物统计学是应用统计学方法来分析和解释生物数据的学科。
本试题集旨在帮助学生掌握生物统计学的基本概念、方法和应用。
以下是一套生物统计学试题及答案。
一、选择题1. 以下哪项不是生物统计学中常用的数据类型?A. 计量数据B. 计数数据C. 定性数据D. 时间序列数据答案:D2. 假设检验中的零假设(Null Hypothesis)通常表示:A. 两个变量之间有显著差异B. 两个变量之间没有显著差异C. 变量之间存在某种关系D. 变量之间不存在任何关系答案:B3. 以下哪个统计量是用来衡量数据集中趋势的?A. 方差B. 标准差C. 平均数D. 极差答案:C二、简答题1. 简述生物统计学中的样本和总体的区别。
答案:样本是指从总体中抽取的一部分个体,用以代表总体进行研究。
总体是指研究对象的全部个体。
样本数据可以用来估计总体参数,但样本并不能完全代表总体。
2. 解释什么是置信区间,并说明其在生物统计学中的应用。
答案:置信区间是一个范围,表示在给定的置信水平下,总体参数的真实值落在这个范围内的概率。
在生物统计学中,置信区间用于表示对样本统计量估计的不确定性,常用于描述实验结果的可靠性。
三、计算题1. 给定一组数据:2, 4, 6, 8, 10。
计算这组数据的平均数、中位数和标准差。
答案:平均数 = (2 + 4 + 6 + 8 + 10) / 5 = 6中位数 = 6(因为数据已经排序,中位数是中间的数值)标准差 = √[(Σ(xi - x̄)²) / (n - 1)] = √[(2(2-6)² +4(4-6)² + 6(6-6)² + 8(8-6)² + 10(10-6)²) / 4] = √[(2*4 +2*4 + 0 + 4*4 + 8*4) / 4] = √[(32 + 32 + 0 + 64 + 128) / 4]= √[256 / 4] = √64 = 8结束语:通过本试题集的练习,希望能够帮助学生更好地理解和应用生物统计学的知识。
生物统计考试试题及答案

生物统计考试试题及答案考试试题:第一部分:选择题1. 下列哪个统计指标可以用于衡量数据的集中趋势?A. 方差B. 标准差C. 平均数D. 相关系数2. 在一组数据中,75%的观测值都小于多少?A. 中位数B. 平均数C. 上四分位数D. 标准差3. 下列哪个统计方法可用于研究两个变量之间的关系?A. 单因素方差分析B. 相关分析C. T检验D. 卡方检验4. 下列哪个分布常用于描述二项试验的结果?A. 正态分布B. 泊松分布C. 几何分布D. 二项分布5. 什么是零假设?A. 假设两个变量之间存在关联B. 假设两个样本均值之间没有差异C. 假设拒绝了原始假设D. 假设样本比例与总体比例相等第二部分:解答题1. 请解释什么是样本均值,并说明其计算公式。
2. 什么是假设检验?请简要介绍假设检验的步骤。
3. 请解释什么是相关系数,并说明其取值范围和含义。
4. 什么是方差分析?请说明方差分析的基本原理及适用范围。
5. 请解释什么是二项试验,并说明如何计算二项试验的概率。
答案:第一部分:选择题1. C. 平均数2. C. 上四分位数3. B. 相关分析4. D. 二项分布5. B. 假设两个样本均值之间没有差异第二部分:解答题1. 样本均值是指由样本数据计算出的平均数,用来估计总体均值。
计算公式为将样本中的所有观测值相加,再除以样本的大小。
2. 假设检验是一种基于样本数据进行统计推断的方法,用于判断一个关于总体参数的假设是否成立。
假设检验的步骤包括:设立原假设和备择假设;选择适当的统计量;设置显著性水平;计算统计量的观察值;根据计算出的观察值与临界值进行比较;做出推断并给出结论。
3. 相关系数是用来衡量两个变量之间线性关系强度和方向的统计指标。
其取值范围为-1到1,当相关系数为正值时,表示两个变量正相关;当相关系数为负值时,表示两个变量负相关;当相关系数接近0时,表示两个变量之间没有线性关系。
4. 方差分析是一种用于比较两个或多个组之间均值差异是否显著的统计方法。
浙大生物统计样卷2010-2011 A答案

余思扬
3100100227
2012 年 6 月
浙江大学 2010–2011 学年 秋冬 学期 《生物统计(学)与实验设计》课程期末考试试卷 — A 卷
Problem 1 (25 points):
One experiment is conducted for studying the influence of plant condition on the content of Nicotine in leaf of tobacco. Two tobacco varieties and two different plant cultivations (fertilization, no fertilization) were arranged, 2 pieces of leaves were sampled for each variety. The obtained experiment data are shown in the following table. 叶片 1 施肥 品种 1 品种 2 9 4 15 12 叶片 2 施肥 5 7 17 11 叶片 1 不施肥 19 14 15 12 叶片 2 不施肥 20 16 17 11
DATA Rice; INPUT Variety $ Density $ Yield @@; DATALINES; A1 B1 8 A1 B2 8 A1 B3 8 A1 B1 7 A1 B2 7 A1 B3 6 A1 B1 6 A1 B2 5 A1 B3 6 A2 B1 9 A2 B2 9 A2 B3 8 A2 B1 7 A2 B2 9 A2 B3 6 A2 B1 8 A2 B2 7 A2 B3 6 ; PROC GLM; CLASS Variety Density; MODEL Yield = Variety | Density; MEANS Variety /TUKEY; MEANS Density /TUKEY; LSMEANS Variety*Density /STDERR PDIFF; RUN;
《生物统计学》试卷与参考答案
《生物统计学》试卷一.判断题(正确的打“√”错误的打“×”,每题2分,共10分)1. 分组时,组距和组数成反比。
( )2. 粮食总产量属于离散型数据。
( )3. 样本标准差的数学期望是总体标准差。
( )4. F 分布的概率密度曲线是对称曲线。
( )5. 在配对数据资料用t 检验比较时,若对数n=13,则查t 表的自由度为12。
( )二. 选择题(每题2分,共10分)1. x ~N (1,9),x 1,x 2,…,x 9是X 的样本,则有( ) A.31-x ~N (0,1)B.11-x ~N (0,1) C.91-x ~N (0,1)D.以上答案均不正确2. 假定我国和美国的居民年龄的方差相同。
现在各自用重复抽样方法抽取本国人口的1%计算平均年龄,则平均年龄的标准误( )A.两者相等B.前者比后者大C.前者比后者小D.不能确定大小3. 设容量为16人的简单随机样本,平均完成工作需时13分钟。
已知总体标准差为3分钟。
若想对完成工作所需时间总体构造一个90%置信区间,则( )A.应用标准正态概率表查出u 值B.应用t 分布表查出t 值C.应用卡方分布表查出卡方值D.应用F 分布表查出F 值 4. 1-α是( )A.置信限B.置信区间C.置信距D.置信水平5. 如检验k (k=3)个样本方差s i 2 (i=1,2,3)是否来源于方差相等的总体,这种检验在统计上称为( )。
A.方差的齐性检验B. t 检验C. F 检验D. u 检验 三. 填空题(每题1分,共10分)1、统计学的3个基本特点: 、 、 。
2、统计资料的特点: 、 、 。
3、统计资料可分为 、和 、两类,后者又可分为 、和 。
4、统计表由 、 、 、 、 组成,通常分为 和 。
5、显著性检验又称 ,是统计学的核心内容。
6、随机实验的每一个可能的结果称为 。
7、通常把α称为显著性水平或置信系数,常用显著性水平有两个,它们是 和 。
生物统计考试题目及答案

生物统计考试题目及答案一、单项选择题(每题2分,共20分)1. 生物统计中,描述数据集中趋势的常用指标是()。
A. 平均值B. 中位数C. 众数D. 标准差答案:A2. 在统计学中,标准差是用来衡量数据的()。
A. 集中程度B. 离散程度C. 相关性D. 正态性答案:B3. 以下哪项不是假设检验中的基本概念()。
A. 零假设B. 备择假设C. 显著性水平D. 置信区间答案:D4. 相关系数的取值范围是()。
A. -1 到 1B. -2 到 2C. 0 到 1D. 0 到 2答案:A5. 以下哪种统计图适合展示分类数据的分布情况()。
A. 折线图B. 条形图C. 散点图D. 箱线图答案:B6. 在回归分析中,回归系数表示的是()。
A. 自变量每变化一个单位,因变量的平均变化量B. 自变量每变化一个单位,因变量的变化量C. 自变量每变化一个单位,因变量的最小变化量D. 自变量每变化一个单位,因变量的最大变化量答案:A7. 以下哪项是描述数据分布形状的统计量()。
A. 平均值B. 标准差C. 偏度D. 峰度答案:C8. 在生物统计中,样本量的大小会影响()。
A. 抽样误差B. 总体参数C. 样本均值D. 样本方差答案:A9. 以下哪项不是方差分析(ANOVA)的基本假设()。
A. 各组样本独立B. 各组样本方差相等C. 各组样本均值相等D. 各组样本服从正态分布答案:C10. 以下哪项是用于比较两个相关样本的统计方法()。
A. 独立样本t检验B. 配对样本t检验C. 方差分析D. 卡方检验答案:B二、多项选择题(每题3分,共15分)11. 下列哪些是生物统计中常用的数据类型()。
A. 定量数据B. 定性数据C. 计数数据D. 等级数据答案:ABCD12. 在进行假设检验时,以下哪些因素会影响p值的大小()。
A. 样本量B. 效应大小C. 显著性水平D. 检验方法答案:ABD13. 以下哪些是描述数据离散程度的统计量()。
生物统计考试题目及答案

生物统计考试题目及答案一、单项选择题(每题2分,共20分)1. 以下哪项不是生物统计学的研究内容?A. 数据收集B. 数据分析C. 统计推断D. 艺术欣赏答案:D2. 在生物统计学中,总体是指:A. 研究对象的全体B. 研究对象的一部分C. 研究对象的样本D. 研究对象的个体答案:A3. 以下哪项是描述性统计中的集中趋势指标?A. 方差B. 标准差C. 平均数D. 极差答案:C4. 假设检验中,如果p值小于显著性水平α,我们通常会:A. 拒绝零假设B. 接受零假设C. 无法判断D. 重新收集数据5. 以下哪项是生物统计学中常用的非参数检验方法?A. t检验B. 方差分析C. 卡方检验D. 秩和检验答案:D6. 相关系数的取值范围是:A. -1到1之间B. -1到0之间C. 0到1之间D. 无法确定答案:A7. 在回归分析中,回归方程的斜率表示:A. 自变量每变化一个单位,因变量的变化量B. 因变量每变化一个单位,自变量的变化量C. 自变量和因变量之间的相关性D. 自变量和因变量之间的因果关系答案:A8. 以下哪项是生物统计学中常用的数据转换方法?A. 对数转换B. 平方转换C. 立方转换D. 所有选项答案:D9. 以下哪项是生物统计学中常用的图形表示方法?B. 条形图C. 箱线图D. 所有选项答案:D10. 在生物统计学中,置信区间的宽度与以下哪项因素无关?A. 样本大小B. 置信水平C. 数据的变异性D. 总体均值答案:D二、多项选择题(每题3分,共15分)1. 以下哪些是生物统计学中常用的数据类型?A. 计数数据B. 测量数据C. 等级数据D. 时间序列数据答案:ABCD2. 在生物统计学中,以下哪些因素会影响样本均值的标准误差?A. 总体标准差B. 样本大小C. 抽样方法D. 总体均值答案:AB3. 以下哪些是生物统计学中常用的参数估计方法?A. 点估计B. 区间估计C. 极大似然估计D. 贝叶斯估计答案:ABCD4. 在生物统计学中,以下哪些因素会影响假设检验的功效?A. 样本大小B. 效应大小C. 显著性水平D. 数据的分布答案:ABCD5. 以下哪些是生物统计学中常用的数据质量控制方法?A. 数据清洗B. 数据转换C. 数据插补D. 数据标准化答案:AC三、简答题(每题10分,共20分)1. 请简述生物统计学中样本量确定的基本原则。
生物统计学试题及答案

生物统计学试题及答案一、选择题(每题3分,共30分)1. 以下哪个不属于生物统计学的基本任务?A. 数据收集B. 数据分析C. 数据可视化D. 数据预测答案:D2. 生物统计学中,下列哪个指标用于衡量样本数据的离散程度?A. 平均数B. 标准差C. 极差D. 方差答案:B3. 在生物统计学中,下列哪个分布适用于描述样本均数的分布?A. 正态分布B. t分布C. 卡方分布D. F分布答案:B4. 在进行生物统计学的假设检验时,下列哪个条件必须满足?A. 样本容量越大越好B. 数据呈正态分布C. 方差相等D. 所有选项都是答案:D5. 生物统计学中,下列哪个方法用于比较两个独立样本的均值差异?A. 成对样本t检验B. 两独立样本t检验C. 方差分析D. 卡方检验答案:B6. 以下哪个方法用于分析生物数据中的相关性?A. 相关分析B. 回归分析C. 主成分分析D. 聚类分析答案:A7. 在生物统计学中,下列哪个分布适用于描述二项分布的总体的标准误?A. 正态分布B. t分布C. 卡方分布D. F分布答案:B8. 以下哪个方法用于检验多个样本之间的方差是否相等?A. F检验B. t检验C. 卡方检验D. 方差分析答案:A9. 在生物统计学中,下列哪个指标用于衡量样本数据的集中趋势?A. 平均数B. 中位数C. 众数D. 所有选项都是答案:D10. 生物统计学中,下列哪个方法用于分析多个变量之间的关系?A. 主成分分析B. 聚类分析C. 回归分析D. 方差分析答案:C二、填空题(每题3分,共30分)1. 生物统计学是研究生物现象的______、______和______的科学。
答案:数据收集、数据分析、数据可视化2. 在生物统计学中,常用的统计量包括______、______、______和______。
答案:平均数、标准差、方差、极差3. 生物统计学中的假设检验包括______检验和______检验。
答案:参数检验、非参数检验4. 在进行生物统计学的假设检验时,需要设定的两个假设分别是______假设和______假设。
《生物统计学》试题A[1]
![《生物统计学》试题A[1]](https://img.taocdn.com/s3/m/440da9792e3f5727a5e96274.png)
《生物统计学》基本知识题
简单相关、偏相关与多元相关
相关系数与决定系数
二、选择题(请将所选答案填在下表中,每题 1 分,共 10 分)
题号
1
2
2
3
4
5
6
7
8
9
10
所选答案
1.二项分布、Poisson 分布、正态分布各有几个参数:( )
A、 (1,1,1 )
B、 (2,2,2)
C、 (2,1,2)
D、 (2,2,1 )
2.第一类错误是下列哪一种概率: ( )
一、填空题
第一章
1.填写下列符号的统计意义:① SS ② S x
③ S2 ④ SP xy。
2.t检验、u检验主要用于____ 组数据的差异显著性检验; F 检验主要用于_____ 组数据的差异显著
性检验。
3.试验误差指由
因素引起的误差,它不可
பைடு நூலகம்
,但可
以
和
。
4.参数是由____计算得到的,统计量是由____计算得到的。
A、它是 x 的 99%置信区间
B、它是总体平均数 的 5%置信区间
C、它是该医学指标的 95%正常值范围
D、它是该医学指标的 99%正常值范围
4. x 、 s 和 s x 同上题,试问区间[ x -2.576 s x , x +2.576 s x ]所代表的含义为:( )
生物统计试题及答案

生物统计试题及答案一、单项选择题(每题2分,共20分)1. 生物统计中,用于描述数据集中趋势的指标是()。
A. 方差B. 标准差C. 平均数D. 极差答案:C2. 以下哪个不是生物统计中的离散程度的度量()。
A. 极差B. 标准差C. 平均数D. 方差答案:C3. 在生物统计中,相关系数的取值范围是()。
A. -1到1B. 0到1C. 0到正无穷D. -1到正无穷答案:A4. 假设检验中,如果P值小于显著性水平α,则()。
A. 拒绝原假设B. 接受原假设C. 无法判断D. 需要重新收集数据答案:A5. 以下哪个不是生物统计中的非参数检验方法()。
A. 卡方检验B. 曼-惠特尼U检验C. 克鲁斯卡尔-瓦利斯检验D. 斯皮尔曼秩相关系数检验答案:A6. 在生物统计中,回归分析用于研究()。
A. 两个变量之间的相关性B. 两个变量之间的因果关系C. 一个变量对另一个变量的影响D. 所有以上选项答案:D7. 生物统计中,方差分析(ANOVA)用于比较()。
A. 两个以上样本的均值差异B. 两个样本的均值差异C. 一个样本的均值与总体均值的差异D. 一个变量的均值与另一个变量的均值的差异答案:A8. 以下哪个是生物统计中的参数检验方法()。
A. 卡方检验B. 曼-惠特尼U检验C. t检验D. 斯皮尔曼秩相关系数检验答案:C9. 在生物统计中,如果一个变量的值增加导致另一个变量的值减少,则这两个变量之间的相关性是()。
A. 正相关B. 负相关C. 无相关D. 无法判断答案:B10. 生物统计中,用于描述数据分布形态的指标是()。
A. 偏度B. 峰度C. 标准差D. 平均数答案:A二、多项选择题(每题3分,共15分)11. 生物统计中,以下哪些是描述数据分布的集中趋势的指标()。
A. 平均数B. 中位数C. 众数D. 方差答案:ABC12. 在生物统计中,以下哪些是描述数据分布的离散程度的指标()。
A. 极差B. 方差C. 标准差D. 偏度答案:ABC13. 以下哪些是生物统计中的参数检验方法()。
(完整word版)浙大生物统计学题库(word文档良心出品)

·《生物统计学》应用题试题库生物统计学应用试题1姓名: 学号: 单位: 得分:一. 某省生物会考满分为100分,平均分71分,标准差12.8分,现定前15%为A等,次25%为B等,问A等、B等的最低分数线各是多少分?二. 为了验证某种“增高”药物的效果,现取某班级男生随机分组后进行对照实三.四.生物统计学应用试题2姓名: 学号: 单位: 得分:一.某省生物会考满分为100分,平均分71分,标准差12.8分,现定前15%为A等,某考生考了88分,问该考生是否达到A等?二.为了检验长跑的体锻效果,某班级12名男生长跑一个月进行前后对比实三.生物统计学应用试题3姓名: 学号: 单位: 得分:一.某省生物摸底考满分为120分,平均分86分,标准差14.8分,现定前後15%为优和差,问优和差的分数线是多少?二.为了检验某减肥药的减肥效果,12名受试者一个月进行前后对比实验,三.理。
生物统计学用于试题4姓名: 学号: 单位: 得分:以上,某学生的一. 美国大学规定獲得獎學金的学生的成績百分位需在P85CEEB=620分,问该生能否獲得獎學金? (注:CEEB=100Z+500)二. 为了检验游泳的体锻效果,某班级12名男生游泳鍛煉一个月进行前后对比三.四.生物统计学应用试题5姓名: 学号: 单位: 得分:一.某省生物会考满分为100分,平均分71分,标准差12.8分,现定前15%为A等,次25%为B等,某考生考了72分,问该考生是否达到B等?二.为了检验长跑的体锻效果,某班级12名男生长跑一个月进行前后对比实验,三.四.生物统计学应用试题6姓名: 学号: 单位: 得分:一.某省生物摸底考满分为120分,平均分71分,标准差15.8分,前15%为A等,次25%为B等,某考生考了92分,问该考生是否达到A等?二.为了检验慢跑的体锻效果,某班级12名男生长跑一个月进行前后对比实验,三.四.生物统计学应用试题7姓名: 学号: 单位: 得分:一.某省生物摸底考满分为120分,平均分86分,标准差14.8分,现定前後15%为优和差,问优和差的分数线各是多少?二.为了检验某治疗高血压药的降压效果,12名受试者一个月进行前后对比实三.四.生物统计学应用试题8姓名: 学号: 单位: 得分:一.2000年托福考试平均550分,标准差30分,美国大学规定獲得獎學金的学生的托福成績百分位需在P以上,问能獲得獎學金最低托福分是多少?90二.为了检验某速记法记英文单词的效果,某班级12名受试生训练一个月进行三.四.生物统计学应用试题9姓名: 学号: 单位: 得分:一.成年女性平均身高158cm,标准差8.8cm,问在1万名成年女性中约有多少人身高超过180cm?二.为了验证某种“增高”药物的效果,现取某校初中男生随机分组后进行对照三.四.生物统计学应用试题10姓名: 学号: 单位: 得分:一.美国英语考试标准分TOEFL=70Z+500,某考生考了627分,求他的成绩百分位。
《生物统计学》试卷A
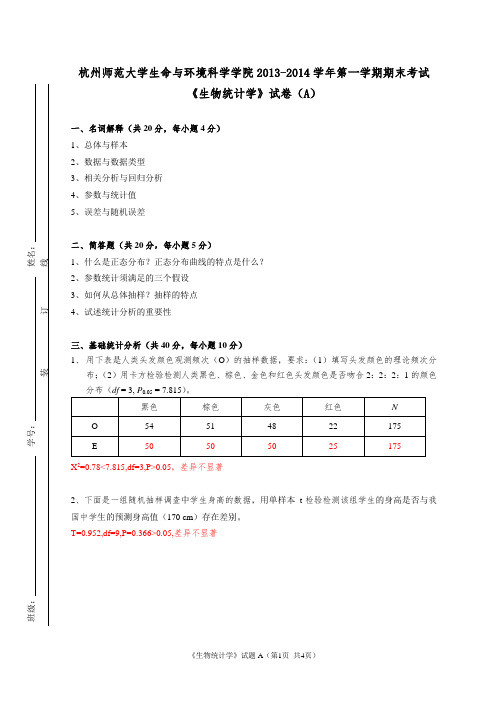
杭州师范大学生命与环境科学学院2013-2014学年第一学期期末考试《生物统计学》试卷(A )一、名词解释(共20分,每小题4分) 1、总体与样本 2、数据与数据类型 3、相关分析与回归分析 4、参数与统计值 5、误差与随机误差二、简答题(共20分,每小题5分)1、什么是正态分布?正态分布曲线的特点是什么?2、参数统计须满足的三个假设3、如何从总体抽样?抽样的特点4、试述统计分析的重要性三、基础统计分析(共40分,每小题10分)1、 用下表是人类头发颜色观测频次(O )的抽样数据,要求:(1)填写头发颜色的理论频次分布;(2)用卡方检验检测人类黑色、棕色、金色和红色头发颜色是否吻合2:2:2:1的颜色分布(df = 3, P 0.05 = 7.815)。
黑色 棕色 灰色 红色 N O 54 51 48 22 175 E50505025175X 2=0.78<7.815,df=3,P>0.05,差异不显著2、下面是一组随机抽样调查中学生身高的数据,用单样本t-检验检测该组学生的身高是否与我国中学生的预测身高值(170 cm )存在差别。
T=0.952,df=9,P=0.366>0.05,差异不显著班级: 学号: 姓名: 装 订 线3、请填写以下未完成的表格并用Mann-Whitney 检验由两位老师分别指导的8名学生是否具有相同的学业表现 A=(1+2+3)/3=2 B=(4+5+6+7+8)/5=6C=(9+10+11+12+13+14)/6=11.5 D=(15+16)/2=15.5分析—非参数检验—两个独立样本 U=24.5,N=16,P=0.4094. 抽测养殖鱼池10次放养记录,结果如表(x:放养量 y :鱼产量。
单位:kg )。
放养量x 鱼产量y 1.9 7.1 2 6.4 2.6 10.4 2.7 10.9 2 7 2.4 10 2.3 7.9 2.2 9.3 3.3 12.8 2.37.5身高(cm )166 170 175 160 173 171 168 159 172 170老师A 老师 B得分 得分等级得分 得分等级A 2 A 2B 6 A 2 B 6 B 6C 11.5 B 6 C 11.5 B 6 C 11.5 C 11.5 C 11.5 C 11.5 D15.5D15.5试求:放养量与鱼产量之间是否存在线性回归关系,若存在请给出线性回归方程。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
Biostatistics 余思扬 3100100227 2012年6月
浙江大学2010–2011学年 秋冬 学期
《生物统计(学)与实验设计》课程期末考试试卷 — A 卷
Problem 1 (25 points):
One experiment is conducted for studying the influence of plant condition on the content of Nicotine in leaf of tobacco. Two tobacco varieties and two different plant cultivations (fertilization, no fertilization) were arranged, 2 pieces of leaves were sampled for each variety. The obtained experiment data are shown in the following table.
叶片1 叶片2 叶片1 叶片2
施肥 施肥 不施肥 不施肥 品种1 9 5 19 20
4 7 14 16 品种2
15 17 15 17
12
11
12
11
(1) What is this experiment design?
This is a typical Crossed Nested Design.
(2) Write out the ANOVA model of this experiment for analysis; define each factor in the model.
()()()
Error
: Leaf : Variety :ion Fertilizat :Mean :2,1 2,1 2,1 2,1 εγβμεαγαβγβαμαn k j i Y ijkn j ik ij j k j i ijkn ====++++++=
(3) For the above ANOVA model, write out the formula of degree freedom and corresponding sum of square
for factors in the model.
Source Degree of Freedom (df) Sum of Squares (SS)
A a-1 = 1 ()∑=∙∙∙∙∙∙∙-a
i i Y Y bcr 12
B b-1 = 1 ()∑=∙∙∙∙∙∙∙-b
j j Y Y acr 1
2
C(B) b(c-1) = 2 ()∑∑==∙∙∙∙∙∙-b j c
k jk Y Y ar 112
AB (a-1)(b-1) = 1 ()∑∑==∙∙∙∙∙∙∙∙∙∙∙∙+--a
i b
j j i ij Y Y Y Y cr 11
2
AC(B) (a-1)b(c-1) = 2 ()∑∑∑===∙∙∙∙∙∙∙∙∙+--a i b j c
k jk ij ijk Y Y Y Y r 111
2
Error
abc(r-1) = 8
()∑∑∑∑====∙-a i b j c k r
n ijk ijkn Y Y
1111
2
Note:
A, B, C and Error are referred to factor α, β, γ and Error ε. And a, b, c and r are the number tested in this design of factor α, β, γ and Error ε.
(4) Write out the SAS program for analysis of this data.
(5) According to the following output of SAS analysis, draw appropriate statistical conclusion.
From the SAS result, we could find that the Pr>F of model is 0.1861>0.05, the model is not reach significant level, need to be adjusted. And from Type III SS we can find that only the factor that significant is fertilization. So the conclusion is in the model, fertilization is significant but the model itself is not reach the significant level, we need to change model. And also we can find in this model, all the interaction factors are missing, so the change of model we can add all the interactions as I write in Question (4) above, and we may get a better result.
Problem 2 (30 points):
One experiment of rice variety is conducted for studying the relationship of yield and planting density. The experiment has 2 varieties (A1, A2) and 3 planting densities 10 (B1), 20 (B2), 30 (B3). The observation data are
It is a typical Two-way Factorial Design.
(2) Write out the ANOVA model for this experiment.
()
Error
: Density : Variety :Mean :3,2,1 3,2,1 2,1 εβμεαββαμαk j i Y ijk ij j i ijk ===+++++=
(3) Can the interaction of variety and planting density be analyzed ?Why?
Yes. Because in this experiment we had three replicates, so we can conduct the analysis of interaction.
(4) Assume the variety is fixed, planting density is random; write out the SAS program to analysis this data.
(5) How to use the SAS to test the difference between B3 and the average of B1 and B2? We should adapt Linear Contrast command in this test: Add this term into PROC:
(6) For this set of experiment data, how to construct a regression model of rice yield on planting density, so
that it can be used to predict rice yield for other planting density.。
