力学专业英语部分翻译(孟庆元)
力学专业英语08

力学专业英语08Lesson 8The planar motion of a rigid bodyThe planar motion of a rigid body occurs when the path of each point lies wholly in a plane, with the planar paths for all points in the body being parallel to each other. A simple example is a wheel mounted on a rotating shaft. The plane of the motion of any point in that system is perpendicular to the axis of the shaft. The restriction to planar motion substantially simplifies the kinematical description in comparison to a general three-dimensional motion. One of the attributes of this simplification is that it will be possible to depict the motion by means of a planar diagram. Without loss of generality, we will always let the xy plane coincide with the plane of motion.By definition, a rigid body is a system of particles that are always at a fixed distance from each other. This geometric restriction means that the movement of any point in the body relative to any other is limited. The existence of such kinematical relations is contrasted by the situation where particles in a system are not rigidly connected. All materials deform when forces are applied to them. The concept of a rigid body is merely a model that we create as an approximation. When we use the rigid body model, we are assuming that the movement of points due to deformation is negligible.Let us consider three arbitrarily selected points A, B and C to becsribed onto a body. The triangle ABC depicted in Figure 1 is useful for characterizing the kinematics of a rigid body motion. To describe where the triangle ABC is situated in space, it wouldbe sufficient to know the coordinates locating point A, and the angle φbetween the x axis and side AB. From this information, the position of a rigid body at any instant is defined by the absolute position of a point in the body and the angular orientation of any line in the body.Our knowledge of the information required to locate the position of a rigid body permits us to describe how the position change with time. Figure 1 depicts the position of triangle ABC at an instant subsequent to the original position of the triangle indicated by the dashed lines. The distances Δx and Δy describe the movement of point A, and Δφdescribes the angle of rotation of line AB. By the definition of a rigid body we know that the angles between all sides of the triangle are constant. It follows then that each line undergoes the same rotation. Hence, angular motion is an overall property of the motion of the body. It is the same regardless of which points in the body are being discussed.Now ,suppose that the movement of point A is specified. In addition to this movement, points B and C may move relative to point A. Because the angular motion is an overall property, the radial lines from point A to points B and C undergo the same rotation. In other words, each point moves in a circular path relative to point A. Therefore, we may concludethatThe motion of a rigid body consists of a superposition of two movements. The first par consists of a movement of all points following the motion of an arbitrarily selected point in the body. The second movement is a rotation about the selected point in which all lines rotate by the same amount.This statement is known as Chasle’s theorem.Special terms are used to describe the motion of rigid bodies.One simple type of motion occurs when every line in the body retains its original orientation, that is , there is no rotation. This is called a translation. Another simple type of motion occurs when one point in the body is fixed in space. Chasle’s theorem states that the motion of the body may be considered to consists solely of a rotation about the fixed point, this is called a pure rotation, Chasle’s theorem may be reworded to state that the motion of a rigid body is the superposition of a translation of an arbitrary point and a pure rotation about that point.Suppose that the motion of point A in the rigid body shown in Figure 2 is known, as is the angular rotation of the body, ω. Point B is an arbitrarily chosen point whose motion we seek. As shown in Fugure 2 the vectors and denote the positions of points A and B respectively. Note that ωis independent of the choice for points A and B, because Chasle’s theorem states that the rotation is the same for all lines in arigid body. The absolute value of ωis called the angular speed of the rigid body.The qualitative insight provided by Chasle’s theorem will enable us to form algebraic relations for determining the velocities and accelerations of points on a rigid body. The relationship between the absolute motions of points A and B is readily formed by referring to the position vectors depicted in figure 2, i.e. In the above equations (2) and (3) ,the velocity and acceleration of point A are denoted by V A and a A respectively. The symbols V B and a B are similarly defined for point B. The vector ωis the angular velocity of the body, and a is the angular acceleration. Both vectors are perpendicular to the plane of motion, so they are parallel to the axis for the rotational portion of the motion. As usual, the angular unit forωand a must beradians.。
工程力学专业英语翻译

When a material exhibits a linear relationship between stress and strain, it is said to be linear elastic. This is an extremely important property of many solid materials, including most metals, plastics, wood, concrete, and ceramics. The linear relationship between stress and strain for a bar in tension can be expressed by the simple equation ζ=Eε (3) in which E is a constant of proportionality known as the modulus of elasticity for the material. 当一种材料的应力与应变表现出线性关系时,我们称这种材 料为线弹性材料。这是许多固体材料的一个极其重要的性质,这 些材料包括大多数金属,塑料,木材,混凝土和陶瓷。对于被拉 伸的梁来说,这种应力与应变之间的线性关系可以用简单方程(3) ζ= Eε 来表示,这里E是一个已知的比例常数,即该材料的弹性模 量。
方程(1) 用于求解在梁中均匀分布的应力问题。它表示了应力 的单位是力除以面积。正如我们在图1中所看到的,当梁被力P拉 伸的时候,生成的应力是拉应力;如果力的方向被颠倒,导致梁 被压缩时,产生的应力被称为压应力。
A necessary condition for Eq.(1) to be valid is that the stress ζ must be uniform over the cross section of the bar. This condition will be realized if the axial force P acts through the centroid of the cross section. When the load P does not act at the centroid, bending of the bar will result, and a more complicated analysis is necessary. At present, however, it is assumed that all axial forces are applied at the centroid of the cross section unless specifically stated to the contrary. Also, unless stated otherwise, it is generally assumed that the weight of the object itself is neglected, as was done when discussing the bar in Fig.1.
力学专业英语资料(一)考研必备

I. Theoretical Mechanics理论力学Gravitational Force, Gravity重力Concurrent Force汇交力, Coplanar Force共面力Force, Torque / Moment扭矩, Couple 约束力,反应,被动力,内力Constraint Force, Reaction, Passive Force, Internal/External Force 静力学直角坐标分量,合力,平行四边形准则Rectangular Components, Resultant Force, Parallelogram Law 1. Statics Composition of Force力的合成, Free Body Diagram隔离体Vector (Magnitude, Direction)矢量, Scalar标量静力平衡平衡方程平衡条件Net Force = 0Static Equilibrium, Equation of Equilibrium, Conditions for EquilibriumNet Torque/Moment = 0Rectilinear MovementMotion of Particle粒子运动Curvilinear Movement运动学 Translation平移2. Kinematics Motion of Rigid Body刚体运动Rotation扭转Linear线性的Velocity速度, Acceleration加速度Angular有角的Momentum, Kinetic Energy, Potential Energy动量,动能,势能动力学Moment of Inertia (Rectangular, Polar), Radius of Gyration惯性矩,回转半径3. Dynamics, Kinetics Centroid (Center of Mass/Gravity)中心Vibration, Oscillation (Free/Forced/Damped/Undamped)振动Simple Harmonic Motion, Period, Frequency简谐振动,周期,频率Pendulum, Centrifugal/Centripetal Force钟摆,离/向心力II. Mechanics of Materials材料力学材料强度 Compressive, Compression压缩1. Strength of Materials Tensile, Tension (Elongation, Extension)伸长Shear, Shearing剪切Linear, Hooke’s LawElastic弹性, Elasticity Non-linearViscoelasticity, Pseudo-elasticity, Super-elasticity粘弹性,拟弹性,超弹性应力应变关系塑性 Perfect Plasticity理想塑性2. Stress-Strain Relation/Behavior Plastic, Plasticity Viscoplasticity, Viscosity黏性, Creeping徐变, RelaxationBrittle脆性, Ductile柔性Work Hardening/Softening, Strain Hardening/Softening弹性模量,弹性系数 Young’s Modulus = Axial Stress / Axial Strain杨氏模量3. Modulus of Elasticity, Elastic Modulus Shear Modulus = Shear Stress / Shear Strain剪切模量Bulk Modulus = Volumetric Stress / Volumetric Strain体积弹性模量破坏理论,失效准则,屈服准则 Maximum Principal Stress Theory最大应力准则4. Failure Theory, Failure Criteria, Yield Criteria Shear Stress TheoryMohr-Coulomb Theory库伦理论Shear Force剪力, Bending Moment弯矩, Flexural Load弯曲荷载梁 Bending Stress弯曲应力, Normal Stress正应力, Shear Stress剪应力 (Horizontal/Longitudinal, Vertical/Transverse)5. Beam Neutral Axis中性轴, Flexure屈曲, Deflection挠曲Cantilever Beam悬臂梁, Simply Supported Beam简支梁, Pin-end, Fixed End固定端Uniformly/Linearly Distributed Load均布荷载, Concentrated Load集中荷载Buckling屈曲系数, Euler’s Equation欧拉方程6. Column 长细比,有效长度,临界荷载,偏心率Slenderness Ratio, Effective Length, Critical Load/Stress, Eccentricity RatioTension弹力, Compression压缩, Uniaxial/Axial Load单轴荷载7. Shaft杆, Rod长杆, Bar Inner/Outer Diameter内/外径Torsion扭转, Torque扭矩, TwistingRadial Distance辐射距离平面应变平面应力双向应力(单轴,三轴)应变能最大/小主应力8. Plane Strain, Plane Stress, Biaxial Stress (Uniaxial, Triaxial), Strain Energy, Major (Minor) Principal Stress Deformation变形, Displacement位移, Deflection偏向Stiffness, Rigidity刚度, Hardness硬度, Flexibility弹性, ComplianceDynamic Loading动力荷载, Cyclic/Fluctuating Loading脉冲荷载, Fatigue疲劳度Thermal Stress热应力/Strain/Deformation, Coefficient of Thermal Expansion热膨胀系数Factor of Safety安全系数, Safety FactorLimit State Design极限状态设计 (Ultimate Limit State极限状态, Serviceability Limit State正常使用极限状态), Probabilistic Design概率设计III. Structural Mechanics, Structural AnalysisRod, Shaft, Bar构件Beam, Girder1. Structural Element Column, PillarPlate, Shell, MembraneShear Wall, Shear Panel2. Truss构架, 3-hinge Arch, Rigid Frame刚性框架 (Joint节点, Pin-Joint, Hinge, Node)3. Statically Determinate静定, Statically Indeterminate超静定, Degree of Static Indeterminacy, N-fold Statically Indeterminate, Degree of Freedom自由度虚功原理 Virtual Displacement, (Matrix) Displacement Method, Stiffness Method4. Virtual Work Principle Virtual Force, (Matrix) Force Method, Flexibility MethodFinite Element MethodIV. Theory of Elasticity(Differential) Equilibrium Equation, Physical Equation, Compatibility/Geometrical EquationBoundary Conditions。
力学专业英语_6
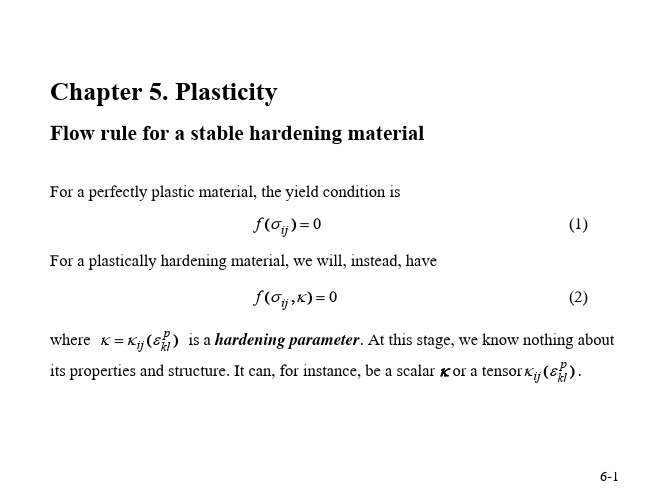
(1)
(2)
p ) is a hardening parameter. At this stage, we know nothing about where κ = κ ij (ε kl p its properties and structure. It can, for instance, be a scalar κ or a tensor κ ij (ε kl ).
Multi-axial case
Extending the above to the multi-axial case, we study a similar load cycle in a multi-axial case (Fig. 3).
Fig.3 Elasto-plastic load cycle, multi-axial case. For this, the analogue of Eq. (4) will be
p (see Fig. 4). This means that must lie behind the border line perpendicular to dε ij
this border line must be tangent to the yield surface. A further consequence is that the yield surface cannot be concave, and since it must be a closed surface enclosing σ ij * , the only possibility left is that it is convex (which in an extreme case can mean that it contains planar surface sections, as, e.g., Tresca’s yield surface). Conclusion (a) above leads directly to the flow rule
理论力学专业词汇中英对照

*静力学statics*运动学kinematics*动力学dynamics*理论力学theoretical mechanics*约束constraint*力系force system*平衡力系balanced force system*刚体rigid body*力偶force couple*力的平移定理theorem of translation of a force*主矢principal vector*主矩principal moment*物体系统(物系)body system (bs)*滑动摩擦sliding friction*摩擦角angle of friction*自锁self-locking*物体的重心the center of gravity of object *轨迹trajectory*速度velocity*加速度acceleration of a particle*运动方程equation of motion*转角angle of rotation*转动方程equation of rotation *角速度angular velocity*角加速度angular acceleration*匀速转动uniform rotation*绝对速度absolute velocity*绝对加速度absolute acceleration*相对速度relative velocity*相对加速度relative acceleration*牵连速度convected velocity*牵连加速度convected acceleration*绝对运动absolute motion*相对运动relative motion*牵连运动convected motion*科氏加速度coriolis acceleration*平面运动方程equations of plane motion *质点particle*动量momentum*冲量impulse*动量矩moment of momentum*功率power*动能kinetic energy*动静法dynamic-static method*虚位移virtual displacements*虚功virtual work。
工程力学常见专业英语词汇总结

空间变量spatial variations
直径diameter
半径radius
必要条件prerequisite
充分条件sufficient condition
质量守恒conservation of mass
动量守恒conservation of momentum
静定问题statically determinate problems
超静定问题statically indeterminate problem
轴向荷载axial load
均布荷载uniform distribution load
集中荷载concentrated load
冲击荷载shock loading
机械能守恒conservation of mechanical energy
能量守恒conservation of energy
守恒定律law of conservation
摩擦friction
内摩擦角friction angle
水平面horizontal plane
假想平面imaginary plane
相互垂直平面mutually perpendicular planes
平面外法向outward normal to the plane
前言preface
后记postscript
附录appendix
连续介质continuousmedium
非连续介质discontinuous medium
周围介质surroundingmedium
气体静力学aerostatics
气体动力学aerodynamics
变形量the amount of deformation
孟庆元工程力学专业英语阅读7
2、 what 作为关系代词,可译为“所…的东西”,“所…的”, 还可根据上下文译成“…的+名词”,“那种+名词”等
What is worrying the world greatly now is a possible shortage of coal, oil, natural gas, or other sources of fuel in the not too distant future.
An element is a simple substance that cannot be broken up into anything simpler.
元素是一种单质,它不能再分任何更简单的物质。
4、that 作为连词引出同位语从句的译法
4.1、同位语从句与主句分译,that译成“即” Energy takes many forms, but all these forms can be reduced
that 作为关系代词,引出定语从句;定语从句可以合译 也可分译,that 的处理方法也随之而异。 3.1、合译时 that 不译,在定语从句之末添“的”字
Power is the rate that mechanical move is performed at. 功率是作机械运动的速率。
3.2、分译时, that 有两种译法: a )重复所代替的名词;b)译成“它” Master is composed of molecules that are composed of atoms. 物质由分子组成,而分子由原子组成。
to the statement that energy is the capacity to do work. 能量具有很多形式,但所有这些形式都可以归纳为这
孟庆元工程力学专业英语阅读3

最好的导体电阻最小,最差的导体电阻最大。
2、某些句子结构须增加关联词
If A is equal to D, A plus B equals D plus B. 若A = D,则A + B = D + B
G Gr
(2)
Equations (1) and (2) relate the strain and stress at the surface of the shaft to the
angle of twist per unit length.
当一个杆件处于纯扭转状态是,其扭转角沿杆件 纵向方向的变化率(即单位长度的扭转角)d / dx是
sections of the bar remain straight and undistorted during twisting, we see that an
interior element situated on the surface of an interior cylinder of radius is also in
6、补充概括性的词 The frequency, wavelength, and speed of sound are closely related. 频率、波长和声速之间是密切相关的。
The resistance of the pipe to the flow of water through it depends upon the length of the pipe, the diameter of the pipe, and the feature of the inside walls (rough or smooth).
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
1、应力和应变应力和应变的概念可以通过考虑一个棱柱形杆的拉伸这样一个简单的方式来说明。
一个棱柱形的杆是一个遍及它的长度方向和直轴都是恒定的横截面。
在这个实例中,假设在杆的两端施加有轴向力F,并且在杆上产生了均匀的伸长或者拉紧。
通过在杆上人工分割出一个垂直于其轴的截面mm,我们可以分离出杆的部分作为自由体【如图1(b)】。
在左端施加有拉力P,在另一个端有一个代表杆上被移除部分作用在仍然保存的那部分的力。
这些力是连续分布在横截面的,类似于静水压力在被淹没表面的连续分布。
力的集度,也就是单位面积上的力,叫做应力,通常是用希腊字母,来表示。
假设应力在横截面上是均匀分布的【如图1(b)】,我们可以很容易的看出它的合力等于集度,乘以杆的横截面积A。
而且,从图1所示的物体的平衡,我们可以看出它的合力与力P必须的大小相等,方向相反。
因此,我们可以得出等式(1)可以作为棱柱形杆上均匀应力的方程。
这个等式表明应力的单位是,力除以面积。
当杆被力P拉伸时,如图所示,产生的应力是拉应力,如果力在方向是相反,使杆被压缩,它们就叫做压应力。
使等式(1)成立的一个必要条件是,应力,必须是均匀分布在杆的横截面上。
如果轴向力P作用在横截面的形心处,那么这个条件就实现了。
当力P没有通过形心时,杆会发生弯曲,这就需要更复杂的分析。
目前,我们假设所有的轴向力都是作用在横截面的形心处,除非有相反情况特别说明。
同样,除非另有说明,一般也假设物体的质量是忽略的,如我们讨论图1的杆一样。
轴向力使杆产生的全部伸长量,用希腊字母δ表示【如图1(a)】,单位长度的伸长量,或者应变,可以用等式来决定。
L是杆的总长。
注意应变ε是一个无量纲的量。
只要应变是在杆的长度方向均匀的,应变就可以从等式(2)中准确获得。
如果杆处于拉伸状态,应变就是拉应变,代表材料的伸长或者延长如果杆处于受压状态,那么应变就是压应变,这也就意味着杆上临近的横截面是互相靠近的。
当材料的应力和应变显示的是线性关系时,也就是线弹性。
这对多数固体材料来说是极其重要的性质,包括多数金属,塑料,木材,混凝土和瓷。
处于拉伸状态下,杆的应力和应变间的线性关系可以用简单的等式来表示。
E是比例常数,叫做材料的弹性模量。
注意E和应力有同样的单位。
在英国科学家托马斯·(1773 ~ 1829)研究杆的弹性行为之后,弹性模量有时也叫做氏模量。
对大多数材料来说,压缩状态下的弹性模量与处于拉伸时的弹性模量的一样的。
2、拉伸应力应变行为一个特殊材料中应力和应变的关系是通过拉伸测试来决定的。
材料的试样通常是圆棒的形式,被安置在测试机上,承受拉力。
当载荷增加时,测量棒上的力和棒的伸长量。
力除以横截面积可以得出棒的应力,伸长量除以伸长发生方向的长度可以得出应变。
通过这种方式,材料的完整应力应变图就可以得到。
图1所示的是结构钢的应力应变图的典型形状,轴向应变显示在水平轴,对应的应力以纵坐标表示为曲线OABCDE。
从O点到A点,应力和应变之间是直接成比例的,图形也是线性的。
过了A点,应力应变间的线性关系就不存在了,因此A点处的应力叫做比例极限。
随着荷载的增加,应变比应力增加的更快,直到在B点,在拉应力没有明显增大的情况下,物体也发生了相当大的伸长。
这种现象叫做材料的屈服,点B处的应力叫做屈服点或者屈服应力。
在区域BC 材料开始具有塑性,棒也开始塑性伸长,伸长量是在比例极限处伸长量的10或者15倍。
在C点,材料开始应变硬化,并且进一步的阻力,阻止载荷的增加。
这样,随着进一步的伸长,应变增加,并且在D点达到最大值,或者极限应变。
过了这一点,棒的拉伸伴随着载荷的减少,试样最后在图上E点断裂。
在棒伸长期间,发生了侧面的收缩,导致棒的横截面积减小。
这个现象在C点之前,对应力应变图没有影响,但是过了这一点,面积的减小对应力的计算值有明显的影响。
棒就会发生明显的颈缩(如图2所示),并且如果颈处狭窄部分的实际横截面积被用于计算σ,将会发现真实的应力应变曲线是虚线CE。
尽管在极限应力达到之后,棒上的总荷载有实际的减小,这个减小是由于面积的减少,而不是材料强度的减小。
在失效点之前,材料实际经受了应力的增加。
然而,为了多数实用目的,常规的应力应变曲线OABCDE是基于试样最初的横截面积,为设计目的提供了令人满意的信息。
图1的图形,画出来是为了表示应力应变曲线的一般特性。
在应力应变曲线的最初区域,材料表现的既有弹性又有线性。
钢材的应力应变图上的从O到A的区域就是很好的例子。
紧接着大的塑性应变,明显屈服点的出现,对于在今天是很普通的结构化金属——钢材来说稍微有点独特。
铝合金从线性到非线性区域是更渐渐的转变。
在失效之前,钢和许多铝合金承受了更大的应变,所以被归类为易延展的。
另一方面,脆性材料在很低的应变时就失效了。
实例包括瓷,铸铁,混凝土,某些金属合金,和玻璃。
3、圆棒的扭转让我们设想一下,一个具有圆形横截面的棒被作用在其末端的力偶扭转(如图1)。
以这种方式加载的棒据称是处于纯扭转。
从考虑对称性可以看出,圆棒的横截面在纵轴方向是作为刚体扭转的,半径依然是直的,横截面是圆形的。
并且,如果棒扭转的总角度比较小的话,棒的长度和半径r都不会改变。
在扭转期间,对应于棒的一端,棒的另一端绕着纵轴会发生扭转。
例如,如果我们把棒的左端看做固定的,那么对应于棒的左端,棒的右端会旋转一个角度。
同时,棒表面的纵向线例如nn,会旋转一个小的角度到位置。
因为扭转,棒表面的矩形单元,例如图中所示的在两个横截面之间相距的单元,被扭转成长菱形。
当一个杆状物承受纯扭转时,扭转角的变化率沿着棒的长度方向是恒定不变的。
这个常数代表单位长度的扭转角,用符合表示。
这样,我们得出,L是轴的长度。
然后,我们可以得到切应变。
作用在单元边线处的切应力有图1所示的方向。
对于线弹性材料,切应力大小是。
等式(1)(2)把杆状物的应变和应力与单位长度的扭转角联系起来。
杆状物部的应力表述用的方式类似于用于杆状物表面的表述方式。
因为棒横截面的半径依然是直的,在扭转时没有扭曲,我们看到位于半径为ρ的圆柱体表面的部单元,是纯剪切并伴随着对应的切应变,应力可以从下述的表达式得出。
这些等式表明,从轴心处切应力和切应变随着径向距离是线性变化的,并且在外表面达到最大值。
作用在横截面的切应力,由等式(3b)给出,伴随着作用在杆状物纵向平面的相等的切应力。
这个结果是从这样一个事实得到的,就是相等的切应力总是存在于相互垂直的平面。
如果材料纵向受剪弱于侧向受剪(例如,木材),受扭杆状物的第一次断裂将会出现在它的纵向表面。
杆状物表面的纯剪切应力的表述等效于,对于杆状物轴扭转45。
的单元上的拉应力和压应力。
如果一种受拉比受剪弱的材料受扭,那么材料将会沿着与轴成45。
的螺旋线处以收缩的方式失效。
通过扭转一支粉笔的方式就可以很容易的演示这种失效。
可以建立施加的扭矩T和产生的扭转角间的关系。
切应力的合力必须静定的等于合扭矩。
作用在单元面积dA上的剪切力是,这个力对于棒轴的力矩是。
在等式(3b)中,力矩等于。
合力矩T是整个横截面上的单元力矩的总和,因此,总和,因此,是圆截面的极惯性矩。
从等式(4)我们可以得到,是单位长度的扭转角,与扭矩T成正比,与乘积,是相反的,是杆的扭转刚度。
4、梁的挠曲一根承受轴横向力的棒叫做梁。
图1中的梁,一端是针状支撑,另一端的滚动支撑,叫做简支梁或者简单的梁。
简支梁的本质特征是在弯曲时梁的两端可以自由转动,但是它不能够横向移动。
另外,梁的一端可以沿轴向自由移动。
一端是嵌入式或者固定,另一端的自由的梁,叫做悬臂梁。
梁的固定端既不可以转动也不可以移动,自由端则可以转动和移动。
梁上的荷载可以分为集中力,例如图1中的力,或者分布载荷,可以表述为沿着梁轴单位距离作用单位力。
轴向力作用于横截面的法向,通过横截面的质心。
剪力??平行于横截面,弯矩作用于平面梁,被叫做合应力。
剪力、弯矩和梁上荷载的关系可以表述为。
这个等式表示,在分布载荷(或者没有载荷)作用于梁上时,弯矩的变化率等于剪力的代数值。
如果梁上作用有集中力,那么在集中力作用点剪力处,将会有突变,或者不连续。
作用在梁侧面的载荷将会引起梁的挠曲。
如图所示,在力作用前,梁的纵轴是直的。
在弯曲后,梁的轴变成了曲线,表现为曲线,让我们假设xy平面是对称与梁的平面,并且所有的载荷都作用在平面。
那么曲线,叫做梁的挠曲线,也会在平面。
从图形的几何形状可以看出,是曲率,等于曲率的半径的倒数。
这样,曲率等于角度在沿着挠曲线测量的长度方面的变换率。
梁挠曲线的基本微分方程可以表述为,是梁从初始位置的挠度。
必须在每个事例中求积分来获得挠度。
这个步骤包括方程的连续积分,作为结果的积分常数从梁的边界条件获得。
应该明白,只有在材料适用于胡克定律并且挠曲线的斜率是很小的时候,方程才是有效。
另一种获得梁挠度的方法是力矩面积法。
这个方法得名于它利用了弯矩图的面积。
当想得到挠度或者梁上一点处的斜率,而不是获得挠曲线的整个方程,这个方法是特别有用的。
作用在横截面上任意一点处的正应力和切应力,可以使用方程,其中是在横截面中性轴方面的第二力矩(或者惯性矩),Q是梁平面面积的第一力矩(或者静态矩)。
可以看出梁外缘处正应力是最大的,在中性轴处为零,在外缘处切应力为零,在中性轴处经常达到最大。
梁上的剪力V和弯矩M经常随着距离变化,距离规定是从它们作用在梁上的横截面处开始的。
当设计一个梁时,非常想知道梁上所以横截面处和的值,提供这方面信息的一个很简便的方法是画一个表达它们沿着梁轴变化的图。
为了画出图,我们把横截面的位置作为横坐标,把对应的剪力或者弯矩的值作为纵坐标。
这样的图像叫做剪力图或者弯矩图。
图1中的简支梁是静定梁中的一种。
这种梁的特征的它所有的反作用力都是由静力平衡方程决定的。
反作用力的数目多于静力平衡方程数目的梁叫做超静定梁。
对于静定梁,我们可以通过求解静力平衡方程快速获得梁的反作用力。
然而,当梁是超静定时,我们不能仅从静力方面求解解决。
取而代之的是,我们必须考虑梁的挠度,并且获得相容方程作为静力方程的补充。
6、刚体的平衡静力学的主要目标是建立一个基本理论,来管理作用在处于平衡状态的物体上的力。
描述阻止物体移动的力的一个手段是自由体受力图,它使物体从周围的事物中隔离出来。
在受力图中,我们展示了施加在物体上的所有力,记住牛顿第三定律告诉我们的,力是物体之间相互作用的结果。
构建受力图的过程帮助我们理解系统的参数是很重要的。
构建自由体受力图的部分任务的为了检查支撑结构,以便我们可以推导出应用在物体上的什么类型的力。
这些力有时叫做约束力,因为它们代表支撑结构约束物体移动的方式。
对这些力的另一个术语是反作用力,因为它们代表支撑结构对物体移动趋势做出反应的方式。
