电磁场与电磁波英文版
《电磁场与电磁波》课程教学大纲

《电磁场与电磁波》课程教学大纲一、课程基本信息课程编码:07S2117B中文名称:电磁场与电磁波英文名称:E1ectromagneticFie1dandE1ectromagneticWave课程类别:专业核心课总学时:48总学分:3适用专业:电子科学与技术专业先修课程:高等数学、大学物理、场论、数学物理方程二、课程性质及目标教学性质:电磁场与电磁波是电子科学与技术专业学生的一门专业核心课程。
通过本课程的学习,要求学生系统地理解电磁场与电磁波的基本概念、基本性质和基本规律,掌握求解电磁场问题的基本方法,为进一步学习其他课程特别是专业课打下基础。
课程目标:1.通过本课程知识的学习,使学生了解电磁场论的发展历程,掌握电磁场论的基本概念、基本性质和基本规律,掌握求解电磁场问题的基本方法,为后续专业课程奠定基础。
引导学生学习科技发展史,树立科技强国意识,感受中国在电子领域的先进成果,激励学生自觉融入到实现中华民族伟大复兴的中国梦进程中。
2.通过本课程知识的学习,使学生掌握电磁场论计算理论的基本方法,并能在具体电子科学与技术专业的具体问题中加以应用。
培养学生解决问题方法的多样性,提高学生数学分析的能力。
3.通过本课程知识的学习,使学生掌握电磁场论分析问题的基本方法,并能在复杂的实际情况中加以应用。
培养学生逻辑思维和创新能力,提高学生设计、开发系统的能力。
不同介质和边界条件对应的场方程形式不同,引导学生用发展的眼光看问题,终身学习,与时俱进,始终拥有先进的理念和较高的职业素养。
I.采用启发式、案例式教学,激发学生主动学习的兴趣,培养学生独立思考、分析问题和解决问题的能力。
2.结合科研生产中的实际例子对课程进行讲解,通过课堂讲解,加强学生对基础知识及基本理论的理解。
3.教学以课堂讲授为主,多媒体辅助教学,提高课堂教学信息量,增强教学的直观性、形象性。
4.通过课内讨论与课外答疑、线下辅导与线上交流相结合的方式,调动学生学习的主观能动性,培养学生的自学能力。
电磁场与电磁波英文教学课件-Ch4 Steady Electric Currents

Electric current, Electromotive force Principle of current continuity, Energy dissipation.
1. Current & Current Density 2. Electromotive Force 3. Principle of Current Continuity 4. Boundary Conditions for Steady Electric Currents 5. Energy Dissipation in Steady Electric Current Fields 6. Electrostatic Simulation
when the impressed electric field is equal but opposite to the electric field produced by the charges on the plates, and the charges will be at rest.
If the conducting medium is connected, the positive charges on the positive electric plate will be moved to the negative electric plate through the conducting medium, while the negative charges on the negative electric plate to the positive electric plate. In this way, the charges on the plates will be decreased, and E < E' . The charges in the source will be moved againthe conductivity, and its unit is S/m. A large
华工电磁场与电磁波全英课Lecture 12

Field and Wave ElectromagneticsField and Wave ElectromagneticsLecture 12李融林R.L. Li4.5Magnetic Fields in Materials and MagneticCircuitsBehavior of Magnetic MaterialsNonmagnetic if(Vacuum)Diamagnetic,if(Silver,Lead,Copper,Water)Paramagnetic, if(Air, Aluminum)Ferromagnetic, if(Cobalt, Nickel, Iron, Silicon iron, Mumetal)The orbiting electrons cause circulating currents and form microscopic magnetic dipoles.In the absence of an external magnetic field the magnetic dipoles of the atoms of most materials have random orientations,resulting in no net magnetic moment.The application of an external magnetic field causes both an alignment of the magnetic moments of the spinning electrons and an induced magnetic moment due to a change in the orbital motion of electrons.4.5.1 Magnetization and Equivalent Current DensitiesWe define a magnetization vector,M ,aswhich is the volume density of magnetic dipole moment. The magnetic dipole moment d m = M dv’will produce a vector magnetic potential(Note thatfor a magnetic dipole of moment .)Usingwe can rewrite d A aswhere V’is the volume of the magnetized material. We now use the vector identityto rewriteThe following vector identity enables us to change the volume integral of the curl of a vector into a surface integral:where F is any vector with continuous first derivatives.Proof: Applying divergence theorem to (F x C), where C is a constant vector, we haveSinceandwe haveWe havewhere is the unit outward normal vector from ds’and S’is the surface bounding the volume V’.The effect of the magnetization vector isequivalent to both a volume current densityand a surface current densityThe problem of finding the magnetic flux density B caused by a given volume density of magnetic dipole moment M is then reduced to finding the equivalent magnetization current densities J m and J ms .Figure 4.5.1The cross-sectionof a magneticmaterial.Example4.5.1Determine the magnetic flux density on the axis of a uniformly magnetized circular cylinder of a magnetic material.The cylinder has a radius b, length L,and axial magnetization .Figure4.5.2A uniform magnetized circular cylinder.SolutionSince Mis a constant,The equivalent magnetization surface current density on the side wallisThe magnet is then like a cylindrical sheet with a surface current density of M(A/m).To find B at P(0,0,z),we consider a differential length dz’with a current andobtain4.5.2 Equivalent Magnetization Charge DensitiesIn a current-free region we may define a scalar magnetic potential V m , from which the magnetic flux density B can be found as . In terms of magnetization vector Mwe may write the scalar magnetic potentialIntegrating this equation over a magnetized body carrying no current, we have We know the gradient of 1/Rwith respect to the primed coordinates isHence(Note thatfor a magnetic dipole of moment.)Recalling the vector identityWe obtainwhere is the outward normal to the surface element ds’.We can conclude that, for field calculation,a magnetized body may be replaced by an equivalentmagnetization surface charge densityρms and an equivalent magnetizationvolume charge densityρmsuch that4.5.3 Boundary Conditions for Magnetostatic FieldsBoundary Condition for BApplying to a small pillbox: the normal component of B is continuous across an interface;For linear media, B 1= µ1H 1 and B 2= µ2H 2Boundary Condition for HSimilarly, applying to a small rectangular closed path, we have orWhere is the outward unit normal from medium 2at the interface .Thus the tangential component of the H field is discontinuous across an interface where a free surface current exists.When the conductivities of both media are finite,current are defined by volume current densities and free surface currents do not exist on the interface.Hence J s =0,and the tangential component of H is continuous across the boundary of almost all physical media;it is discontinuous only when an interface with an ideal perfect conductor or a superconductor is assumed.Figure 4.5.3Boundary condition for H .Example 4.5.2Two magnetic media with permeabilities µ1and µ2have a common boundary,as shown in Figure 4.5.4.Determine the magnitude and the direction of the magnetic field intensity at point P 2in medium 2.SolutionFigure 4.5.4Boundary conditions for Hand B.The desired unknown quantities are H 2andα2.Continuity of the normal component of BfieldrequiresThe tangential component of H field is continuous.WehaveDivision of the second equation by the first equation giveswhich describes the refraction property of the magnetic field.If medium 1is nonmagnetic and medium 2is ferromagnetic,then µ2>>µ1and,α2will be nearly 90degrees.If medium 1is ferromagnetic medium and medium 2is air,then α2will be nearly zero.We obtainThe magnitude of H 2isExample4.5.3Find the image currents of a long straight line current I above an interface between two magnetic media with permeabilitiesµ1andµ2SolutionFigure4.5.5A long straight line current I above an interface between two magnetic media(a);Image current for medium1(b)and image current for medium2(c).Since there is no surface current at the interface which leads toUsing yieldsororSolving the equations givesForForFigure4.5.6Magnetic field lines of a long straight line current in two media.Example 4.5.4Sketch the magnetic flux lines both inside and outside a cylindrical bar magnet having a uniform axial magnetization.Figure 4.5.2A uniform magnetizedcircular cylinder.SolutionWe know that the problem of a cylindrical barmagnet could be replaced by that of amagnetization current sheet having a surfacecurrent density J ms =M 0(J m =0).It is obvious from above equations that the magnetic flux density along the axis at the end faces of the magnet is less than that at the center.Fromwe getOn the side of the magnet there is a surface current given byHence according tothe axial component of B changes by anamount equal to µ0M 0.It must be remarked that while H =B/µ0outside the magnet,H and B inside themagnet are far from being proportionalvectors in the same direction.From H =B /µ0–M ,and the fact that B/µ0along theaxis inside is less than M 0,we observe thatH and B are in opposite directions alongthe axis inside.For a long,thin magnet,L>>b,B p0~µ0M 0.From H =B/µ0–M,weobtain H p0~0.Figure 4.5.7Magnetic flux linesaround a cylindrical bar magnet.4.5.4 Magnetic CircuitsThe curl equation for magnetic fields in materials iswhere J(A/m2) is the volume density of free current.The corresponding integral form is obtained asorwhich is the Ampere’s circuital law in materials,where C is the contour bounding the surface S and I is the total free current passing through S. Ampere’s circuital law states that the circulation of the magnetic field intensity around any closed path is equal to the free current flowing through the surface bounded by the path.Ampere’s circuital law is useful on determining the magnetic field in magnetic circuits.The quantity V mmf (=NI )is called a magnetomotive force (mmf).We define reluctance R as the ratio of the magnetic voltage to the flux ;thusAmpere’s Law for an N-turn magnetic circuit becomesSimilar to Kirchhoff’s voltage law,we may write,for any closed path in a magneticcircuit,Around a closed path in a magnetic circuit the algebraic sum of ampere-turns is equal to the algebraic sum of the products of the reluctances andfluxes.Kirchhoff’s current law for a junction is consequence of. Similarly leads to. Thus we havewhich states that the algebraic sum of all the magnetic fluxes flowing out of a junction in a magnetic circuit is zero.Example 4.5.5N turns of wire are wound around a toroidal core of aferromagnetic material with permeabilityµ.Determine Bf ,in the ferromagneticcore;Hf in the core;and Hgin the airgap.Figure4.5.8Coil on ferromagnetictoroid with air gap.Applying Ampere’s circuital lawSolutionIf flux leakage is neglected,the same totalflux will flow in both the ferromagnetic coreand in the air gap.If the fringing effect ofthe flux in the air gap is also neglected,themagnetic flux density B in both the core andthe air gap will also be the same.However,because of the different permeabilities,themagnetic field intensities in both parts willbe different.Wehavewhere the f and g denote ferromagnetic and gap, respectively.In the ferromagnetic core,and, in the air gap,Ampere’s law yieldsWe haveSimilarlyIf the radius of the cross section of the core is much smaller than the mean radius of the toroid,the magnetic flux density B in the core is approximately constant,and the magnetic flux in the circuitiswhere S is the cross-sectional area of the core.where R f and R g are the reluctances of the ferromagnetic core and the air gap, respectively .Figure 4.5.9Equivalent magneticcircuit and analogous electric circuitfor toroidal coil with air gap.Therefore,or,mfmfThe reluctances are:The two loop equations areSolving these simultaneous equations, we haveExample 4.5.6Find the flux linked with coil N 1in a magnetic circuit shown in Figure 4.5.10.Figure 4.5.10A magnetic circuit.Solution.HomeworkProblems P.6-26 and P.6-27References &Acknowledgements1.M.J.Rhee’s Lectures on Electromagnetic Theory,2005.2.W.H.Hayt,J.A.Buck,Engineering Electromagnetics,7th Ed.,McGraw-Hill,2006.3.U.S.Inan,A.S.Inan,Engineering Electromagnetics,AddisonWesley Longman,2000.4. D.Cheng,Field and Wave Electromagnetics,Second Edition,Addison Wesley,1992.。
电磁场与电磁波:4 Steady Electric Current_zmh(英文)

dUe e E Ue Relaxation-time Approximation
dt
m
:第一项为驱动项,第二项为耗散项。
Under steady conditions, we have:
eE Ue 0
m
e
Ue m E
Mobility:
e
e
m
4.2.2 Conduction Current Density
1
4.1 Introduction
Electrostatics: dv/dt=0 I=0
Steady Electric Current(自 由电荷产生的电流): dv/dt=0 I≠0
2
4.2 Nature of Current and Current Density
4.2.1 Conduction (传导)Current 4.2.2 Conduction Current Density 4.2.3 Convection (运流)Current 4.2.4 Convection Current Density
V 0
( Ne)
0
8
9
10
4.3 Resistance of a Conductor
R U l E dl I sJ ds
(1) Parallel-plate resistor R l
S
(2) A spherical resistor R b a
4ab
(3) Concentric cylindrical conductors resistor
G-R (Depletion Region) D-R
(Quasi-neutral region)
Jn 0, J p 0, but (Jn J p ) 0
电磁场与电磁波词汇

【一画】一致性几何绕射理论UTD (Uniform Geometrical Theory of Diffraction)【二画】二端口网络two port network二重傅立叶级数double Fourier series入射场incident field入射波incident wave几何绕射理论GTD (Geometrical Theory of Diffraction)【三画】小波wavelet【四画】无功功率reactive power无限(界)区域unbound region无源网络passive network互易性reciprocity互阻抗mutual impedance互耦合mutual coupling互连interconnect天线antennas天线方向性图pattern of antenna匹配负载matched load孔aperture孔(缝)隙天线aperture antennas内阻抗internal impedance介电常数permittivity介质dielectric介质波导dielectric guide介质损耗dielectric loss介质损耗角dielectric loss angle介电常数dielectric constant反射reflection反射系数reflection coefficient分离变量法separation of variables【五画】主模dominant mode正交性orthogonality正弦的sinusoidal右手定则right hand rule平行板波导parallel plate waveguide平面波plane wave功率密度density of power功率流(通量)密度density of power flux 布魯斯特角Brewster angle本征值eigen value本征函数eigen function边值问题boundary value problem四端口网络four terminal network矢量位vector potential电压voltage电压源voltage source电导率conductivity电流元current element电流密度electric current density电荷守恒定律law of conservation of charge 电荷密度electric charge density电容器capacitor电路尺寸circuit dimension电路元件circuit element电场强度electric field intensity电偶极子electric dipole电磁兼容electromagnetic compatibility矢量vector矢径radius vector失真distortions平移translation击穿功率breakdown power节点node【六画】安培电流定律Ampere’s circuital law传播常数propagation constant亥姆霍兹方程Helmholtz equation动态场dynamic field共轭问题conjugate problem共面波导coplanar waveguide (CPW)有限区域finite region有源网络active network有耗介质lossy dielectric导纳率admittivity同轴线coaxial line全反射total reflection全透射total transmission各向同性物质isotropic matter各向异性nonisotropy行波traveling wave光纤optic fiber色散dispersion网格mesh全向天线omnidirectional antennas阵列arrays【七画】串扰cross-talk回波echo良导体good conductor均匀平面波uniform plane wave均匀传输线uniform transmission line近场near-field麦克斯韦方程Maxwell equation克希荷夫电流定律Kirchhoff’s current law 环行器circulator贝塞尔函数Bessel function时谐time harmonic时延time delay位移电流electric displacement current芯片chip芯片组chipset远场far-field【八画】变分法variational method定向耦合器directional coupler取向orientation法拉第感应定律Faraday’s law of induction 实部real part空间分量spatial components波导waveguide波导波长guide wave length波导相速度guide phase velocity波阻抗wave impedance波函数wave function波数wave number泊松方程Poisson’s equation拉普拉斯方程Laplace’s equation坡印亭矢量Poynting vector奇异性singularity阻抗矩阵impedance matrix表面电阻surface resistance表面阻抗surface impedance表面波surface wave直角坐标rectangular coordinate极化电流polarization current极点pole非均匀媒质inhomogeneous media非可逆器件nonreciprocal devices固有(本征)阻抗intrinsic impedance单位矢量unit vector单位法线unit normal单位切线unit tangent单极天线monopole antenna单模single mode环行器circulator驻波standing wave驻波比standing wave ratio直流偏置DC bias【九画】标量位scalar potential品质因子quality factor差分法difference method矩量法method of moment洛伦兹互易定理Lorentz reciprocity theorem 屏蔽shield带状线stripline标量格林定理scalar Green’s theorem面积分surface integral相对磁导率relative permeability相位常数phase constant相移器phase shifter相速度phase velocity红外频谱infra-red frequency spectrum矩形波导rectangular waveguide柱面坐标cylindrical coordinates脉冲函数impulse function复介电常数complex permittivity复功率密度complex power density复磁导率complex permeability复矢量波动方程complex vector wave equation 贴片patch信号完整性signal integrity信道channel寄生效应parasite effect指向天线directional antennas喇叭天线horn antennas【十画】准静态quasi-static旁路电流shunt current高阶模high order mode高斯定律Gauss law格林函数Green’s functi on连续性方程equation of continuity耗散电流dissipative current耗散功率dissipative power偶极子dipole脊形波导ridge waveguide径向波导radial waveguide径向波radial wave径向模radial mode能量守恒conservation of energy能量储存energy storage能量密度power density衰减常数attenuation constant特性阻抗characteristic impedance特征值characteristic value特解particular solution勒让德多项式Legendre polynomial积分方程integral equation涂层coating谐振resonance谐振长度resonance length【十一画】混合模hybrid mode部分填充波导partially filled waveguide 递推公式recurrence formula探针馈电probe feed接头junction基本单位fundamental unit理想介质perfect dielectric理想导体perfect conductor唯一性uniqueness虚部imaginary part透射波transmission wave透射系数transmission coefficient球形腔spherical cavity球面波spherical wave球面坐标spherical coordinate终端termination终端电压terminal voltage射频radio frequency探针probe【十二画】涡旋vortices散度方程divergence equation散射scattering散杂电容stray capacitance散射矩阵scattering matrix斯托克斯定理Stoke’s theorem斯涅尔折射定律Snell’s law of refraction阴影区shadow region超越方程transcendental equation超增益天线supergain antenna喇叭horn幅角argument最速下降法method of steepest descent趋肤效应skin effect趋肤深度skin depth微扰法perturbational method等相面equi-phase surface等幅面equi-amplitude surface等效原理equivalence principle短路板shorting plate短截线stub傅立叶级数Fourier series傅立叶变换Fourier transformation第一类贝塞耳函数Bessel function of the first kind第二类汉克尔函数Hankel function of the second kind 解析函数analytic function激励excitation集中参数元件lumped-element场方程field equation场源field source场量field quantity遥感remote sensing振荡器oscillators滤波器filter【十三画】隔离器isolator雷达反射截面radar cross section (RCS)损耗角loss angle感应电流induced current感应场induction field圆波导circular waveguide圆极化circularly polarized圆柱腔circular cavity铁磁性ferromagnetic铁氧体陶瓷ferrite ceramics传导电流conducting current传导损耗conduction loss传播常数propagation constant传播模式propagation mode传输线模式transmission line mode传输矩阵transmission matrix零点Zero静态场static field算子operator输入阻抗input impedance椭圆极化elliptically polarized微带microstrip微波microwave微波单片集成电路microwave monolithicintegrated circuit MMIC毫米波单片集成电路millimeter wave monolithic integrated circuit M3IC 【十四画】漏电电流leakage current渐进表示式asymptotic expression模式mode模式展开mode expansion模式函数mode模式图mode pattern截止波长cut off wavelength截止频率cut off frequency鞍点saddle频谱spectrum线性极化linearly polarized线积分line integral磁矢量位magnetic vector potential磁通magnetic flux磁场强度magnetic intensity磁矩magnetic moment磁损耗角magnetic loss angle磁滞损耗magnetic hysteresis磁导率permeability【十五画】辐射radiate增益gain横电场transverse electric field横电磁波transverse electromagnetic wave 劈wedge【十六画】雕落场evanescent field雕落模式evanescent mode霍尔效应Hall effect辐射电阻radiation resistance辐射电导radiation conductance辐射功率radiation power辐射方向性图radiation pattern谱域方法spectral method【十七画以上】瞬时量insaneous quantity镜像image峰值peak valueδ函数delta function。
电磁场与电磁波课程简介(英文版)

Course code: 131300112Title: Electromagnetic Field and Electromagnetic waveCredit rating: 3.5Time: Semester SixBrief description:This course makes students master the theorem and the physical meaning of the Maxwell equations and mathematical expressions. It includes the electromagnetic field and electromagnetic wave. Part one is the electromagnetic field. It makes students to learn using the method of vector analysis on the basis of electromagnetism course to describe the essential physical concept of electrostatic field and constant magnetic field, and giving the basic law of electromagnetic field based on summarizing the basic law of experiment, and studying the method to solve problems in the static field. Electromagnetic wave part mainly introduces about the propagation rules of electromagnetic waves in a variety of media and the basic theory of antenna.Syllabus1.Vector analysisVector algebra, three kinds of commonly used orthogonal coordinate system, the gradient of a scalar field, vector field flux and the divergence of the vector field of circulation and curl, irrotational field and solenoidal field, Laplace operation with green's theorem.2.The basic rule of electromagnetic fieldCharge conservation law, the basic rule of electrostatic field in vacuum, the basic law of constant magnetic field in vacuum, electromagnetic properties of medium, the law of electromagnetic induction and the displacement current, Maxwell's equations, boundary conditions of electromagnetic field.3. Static electromagnetic field and its solution of boundary value problemsElectrostatic field analysis, a conductive medium constant electric field analysis, constant magnetic field analysis, the boundary value problem ofa static field and uniqueness theorem of solution, image method, separation variable method, finite difference method.4. Time-varying electromagnetic fieldWave equation, the electromagnetic field of a function, the law of conservation of electromagnetic energy, the uniquenesstheorem ,time-harmonic electromagnetic field5. Uniform plane wave propagation in unbounded spaceIdeal medium uniform plane wave, polarization of electromagnetic wave, Uniform plane wave propagation in conductive medium, Uniform plane wave propagation in anisotropic medium6. Uniform plane wave reflection and transmissionThe uniform plane wave vertical incidence on the plane, the uniform plane wave vertical incidence in multilayer dielectric plane, the uniform plane wave oblique incidence in the ideal dielectric plane, the uniform plane wave oblique incidence in the ideal conductor plane7. Guided electromagnetic waveIntroduction to guide line of electromagnetic wave, rectangular waveguide, cylindrical waveguide, coaxial waveguide, and resonant cavity8. The electromagnetic radiationRetarded potential, Electric dipole radiationRecommended TextbooksXie Chu-fang and Rao Ke-jin, Electromagnetic Field and Electromagnetic Waves, Higher Education Press, 2006。
电磁场与电磁波英文版第二版教学设计
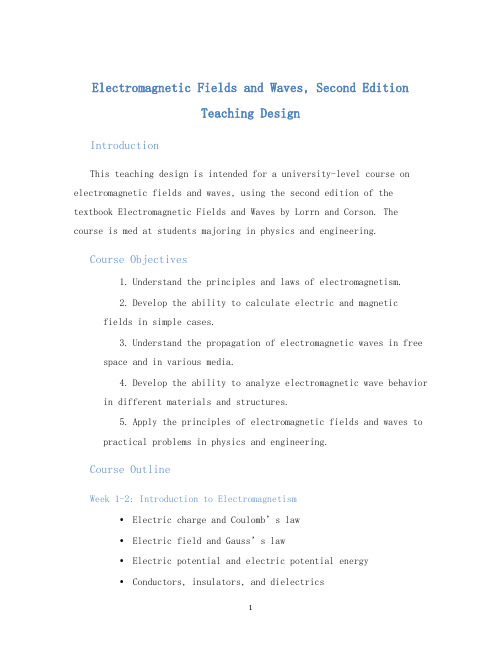
Electromagnetic Fields and Waves, Second EditionTeaching DesignIntroductionThis teaching design is intended for a university-level course on electromagnetic fields and waves, using the second edition of the textbook Electromagnetic Fields and Waves by Lorrn and Corson. The course is med at students majoring in physics and engineering.Course Objectives1.Understand the principles and laws of electromagnetism.2.Develop the ability to calculate electric and magneticfields in simple cases.3.Understand the propagation of electromagnetic waves in freespace and in various media.4.Develop the ability to analyze electromagnetic wave behaviorin different materials and structures.5.Apply the principles of electromagnetic fields and waves topractical problems in physics and engineering.Course OutlineWeek 1-2: Introduction to Electromagnetism•Electric charge and Coulomb’s law•Electric field and Gauss’s law•Electric potential and electric potential energy•Conductors, insulators, and dielectrics•Capacitance and electric energy storageWeek 3-4: Magnetic Fields and Forces•Magnetic field and Ampere’s law•Magnetism and magnetic materials•Magnetic forces on charged particles•Magnetic forces on current-carrying wires and loops•Magnetic energy and inductanceWeek 5-6: Time-Varying Fields and Maxwell’s Equations•Electromagnetic induction and Faraday’s law•Lenz’s law and electromagnetic forces•Maxwell’s equations and electromagnetic waves•Electromagnetic wave solutions and polarization•Reflection, refraction, and standing wavesWeek 7-8: Waveguides and Transmission Lines•Waveguides and resonators•Transmission lines and impedance•Smith charts and matching networks•Radiation and antennas•Applications to wireless communicationsTeaching MethodologyThe course will consist of lectures, problem sets, and laboratory experiments. Lectures will cover the fundamental principles and concepts of electromagnetic fields and waves, and will be supplemented by numerical examples and demonstrations. Problem sets will providestudents with opportunities to develop their problem-solving skills and gn deeper insights into concepts. Laboratory experiments will allow students to apply their theoretical knowledge to real-world situations and gn practical experience with electromagnetic equipment and measurement techniques.AssessmentAssessment will be based on the following criteria:•Short quizzes on lecture topics (10%)•Problem sets on course content (30%)•Laboratory reports on experiment results (30%)•Midterm exam on course material (15%)•Final exam on course material (15%)The grading scale will be based on the following scheme:•A: 90-100%•B: 80-89%•C: 70-79%•D: 60-69%•F: below 60%ConclusionThis teaching design provides an overview of the topics, objectives, and assessment for a course on electromagnetic fields and waves, using the second edition of the textbook Electromagnetic Fields and Waves by Lorrn and Corson. The course will cover the fundamental principles and laws of electromagnetism, as well as the propagation and behavior ofelectromagnetic waves in various media and structures. The course will use a combination of lectures, problem sets, and laboratory experiments to enhance students’ understanding and mastery of the subject matter.。
电磁场与电磁波英文教学课件-Ch

A loop coil with N turns the magnetic flux linkage with the current
is = N , and the inductance of the loop coil with N turns is
z
L N
II
l1
I1
l2
I2 Suppose we have two loop currents,
S
(
E)
B t
dS
0
Since the equation holds for any area S, the integrand must be
zero, so that
E B t
E B t
which is called the differential form of law of electromagnetic induction, and it means that the negative time rate of change of the magnetic flux density at a point is equal to the curl of the timevariable electric field intensity at that point.
flux linkage with the current I, and it is denoted as . The ratio of
to I is denoted by L, hence L
I
It is called the inductance of the circuit, with the unit henry (H), and the inductance can be also considered as the magnetic flux linkage per unit current.
大学英文版电磁学讲义1-2

Chapter 1 History and Perspective 历史与前景
The electromagnetic interaction(电磁相互作用) is one of the fundamental interactions(基本相互作用) of the physical world. Interaction: atoms and molecules. Phenomenon: sunshine, lightning, rainbows. Technology: communication with NASA's planetary probes(行星探测器), electromagnetic medical imaging(医学成象), computer electronics.
Additi i A y B y j A z B z k
Multiplication: 乘法
Chapter 1 History and Perspective 历史与前景 Modern theory of gravity: Einstein's general relativity(广义相对论).
Elementary Charges, Photons, and QED 基本电荷, 光子和量子电动力学
1.1 Brief history of the science of electromagnetism 电磁科学简史
Electric and magnetic phenomena have been known for millenia. Ancient Greece. Amber(琥珀) rubbed with animal fur can attract small bits of matter. The force between natural magnets(磁铁), ferromagnetism(铁磁性). Early part of the scientific revolution in 1600. Gilbert. an important book.
电磁场与电磁波课程简介(英文版)

电磁场与电磁波课程简介(英文版)Course code: 131300112Title: Electromagnetic Field and Electromagnetic waveCredit rating: 3.5Time: Semester SixBrief description:This course makes students master the theorem and the physical meaning of the Maxwell equations and mathematical expressions. It includes the electromagnetic field and electromagnetic wave. Part one is the electromagnetic field. It makes students to learn using the method of vector analysis on the basis of electromagnetism course to describe the essential physical concept of electrostatic field and constant magnetic field, and giving the basic law of electromagnetic field based on summarizing the basic law of experiment, and studying the method to solve problems in the static field. Electromagnetic wave part mainly introduces about the propagation rules of electromagnetic waves in a variety of media and the basic theory of antenna.Syllabus1.Vector analysisVector algebra, three kinds of commonly used orthogonal coordinate system, the gradient of a scalar field, vector field flux and the divergence of the vector field of circulation and curl, irrotational field and solenoidal field, Laplace operation with green's theorem.2.The basic rule of electromagnetic fieldCharge conservation law, the basic rule of electrostatic field in vacuum, the basic law of constant magnetic field in vacuum,electromagnetic properties of medium, the law of electromagnetic induction and the displacement current, Maxwell's equations, boundary conditions of electromagnetic field.3. Static electromagnetic field and its solution of boundary value problemsElectrostatic field analysis, a conductive medium constant electric field analysis, constant magnetic field analysis, the boundary value problem ofa static field and uniqueness theorem of solution, image method, separation variable method, finite difference method.4. Time-varying electromagnetic fieldWave equation, the electromagnetic field of a function, the law of conservation of electromagnetic energy, the uniqueness theorem ,time-harmonic electromagnetic field5. Uniform plane wave propagation in unbounded spaceIdeal medium uniform plane wave, polarization of electromagnetic wave, Uniform plane wave propagation in conductive medium, Uniform plane wave propagation in anisotropic medium6. Uniform plane wave reflection and transmissionThe uniform plane wave vertical incidence on the plane, the uniform plane wave vertical incidence in multilayer dielectric plane, the uniform plane wave oblique incidence in the ideal dielectric plane, the uniform plane wave oblique incidence in the ideal conductor plane7. Guided electromagnetic waveIntroduction to guide line of electromagnetic wave, rectangular waveguide, cylindrical waveguide, coaxial waveguide, and resonant cavity8. The electromagnetic radiationRetarded potential, Electric dipole radiationRecommended TextbooksXie Chu-fang and Rao Ke-jin, Electromagnetic Field and Electromagnetic Waves, Higher Education Press, 2006。
电磁场与电磁波英文参考书.

Pentech Press
参考书
Electromagnetics
Seely, S.
Poularikas, A. D.
Marcel Dekker, Inc.
1979
研究生
Methods in Electromagnetic Wave Propagation
Jones, D. S.
Intext Educational Publishers
1971
参考书
Analysis and computation of Electric and Magnetic Field Problems
Binns, K. J.
Lawrenson, P. L.
Pergamon
1973
参考书
Electromagnetics (2nd)
Johnk, Cafl T. A.
John Wiley & Sons
1988
本科生
The Electromagnetic Field
Shadowitz, A.
DoverPublications
1988
本科生
Advanced Engineering Electromagnetics
Balanis, C. A.
Sihvola, A. H.
Viitanen, A. J.
Lindell,I.V.
Tretyakov,S.A.
Artech House
1994
参考书
Essentials of Electromagnetism
Dugdale, D.
AIP Pand Waves in Communication Electronics
电磁场与电磁波(英文版)ppt课件

Electrostatic field and steady magnetic field are independent of each other,
and may be investigated separately.静电场与恒定磁场相互无关、彼此独立,
可以分别进行研究
5
Entity 物质属性
Electromagnetic Fields and Waves
Rugui Yang
Higher Education Press Higher Education Electronic and
Video Press
1
The physical understanding is emphasized instead of the mathematical derivation.
The magnetic fields unchanging with time are called steady magnetic
fields. 不随时间变化的磁场称为恒定磁场。
4
Electromagnetic Wave电磁波
If the charge and the current vary with time, the electric field and magnetic field they produce will be functions of time.如果电荷及电流均随时间改变,它 们产生的电场及磁场也是随时变化的
Time-varying electric field and magnetic field must co-exist and have definite relation to each other, leading to a time-varying electromagnetic field. 时变的电场与时变的磁场可以相互转化,两者不可分割,它们构成统一的时 变电磁场
电磁场与电磁波英文教学课件-Contents

in Rectangular Coordinates 4. Method of Separation of Variables
in Cylindrical Coordinates 5. Method of Separation of Variables
in Spherical Coordinates
Preface Chapter 1 Vector Analysis
1. Directional Derivative and Gradient of Scalar Fields 2. Flux and Divergence of Vector Fields 3. Circulation and Curl of Vector Fields 4. Solenoidal and Irrotational Fields 5. Green’s Theorems 6. Uniqueness Theorem for Vector Fields 7. Helmholtz’s Theorem 8. Orthogonal Curvilinear Coordinates
6. Principle of Duality 7. Principle of Image 8. Principle of Reciprocity 9. Huygens’ Principle 10. Radiation by Aperture Antennas
电磁场与电磁波(英文版)ppt课件

The magnetic fields unchanging with time are called steady magnetic
fields. 不随时间变化的磁场称为恒定磁场。
4
Electromagnetic Wave电磁波
If the charge and the current vary with time, the electric field and magnetic field they produce will be functions of time.如果电荷及电流均随时间改变,它 们产生的电场及磁场也是随时变化的
为了研究方便起见,我们先介绍真空中的电磁场,然后再讨论媒质中的电 磁场。
7
Field & Source 场与源
Electric charges and currents are the only sources for producing electromagnetic fields. Up to now, no magnetic charge or magnetic current has been found to exist in nature.电荷及电流是产生电磁场惟一的源。至今,人们 尚未发现自然界中存在磁荷及磁流。
Investigation on and its source is a fundamental subject. We will introduce a number of mathematical equations to describe the relationship between the field and the source, as well as between the field and the media.研究场与源的关系是电磁理论的基本问题之一。我们将 要详述场与源,以及场与媒质之间的关系,并且给予严格的数学描述。 8
电磁场与电磁波1

– Suppose at ωt=0, the traveling wave equation becomes A cos( β z ) f ( z , t ) = A cos(ω t − β z ) – Distance zo for one period can be determined by zo
The Shanghai Transrapid Maglev Line: speed up to 430 km/hr.
Japanese Bullet Train
What are Fields?
• Gravitational Field
• Magnetic Field
• Electric Field
1.4 Orthogonal Coordinate Systems
1.4 Orthogonal Coordinate Systems
1.4 Orthogonal Coordinate Systems
1.5 Vector Differential Operators
1.5 Vector Differential Operators
A cos( β z )
– Suppose at ωt=π/4 , the traveling equation The wave moved a distance of π /4β becomes in π /4ω seond. What is the velocity?
A cos(π / 4 − β z )
1.5 Vector Differential Operators
1.5 Vector Differential Operators
1.5 Vector Differential Operators
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
1. Directional Derivative & Gradient
The directional derivative of a scalar at a point indicates the spatial rate of change of the scalar at the point in a certain direction. l
Δl
P
P
of scalar l P at point P in the direction of l is defined as
The directional derivative
l
lim
P
( P) ( P)
Δl
Δl 0
The gradient is a vector. The magnitude幅度 of the gradient of a scalar field at a point is the maximum directional derivative at the point, and its direction is that in which the directional derivative will
be maximum.
In rectangular coordinate system直角坐标系, the gradient of a scalar field can be expressed as
grad e x
ey ez x y z
Where “grad” is the observation of the word “gradient”. In rectangular coordinate system, the operator算符 is denoted as
In rectangular coordinates, the divergence can be expressed as
Ax Ay Az divA x y z
Using the operator , the divergence can be written as
divA A
The direction of a closed surface is defined as the outward normal on the closed surface. Hence, if there is a source in a closed surface, the flux of the vectors must be positive; conversely, if there is a sink, the flux of the vectors will be negative. The source a positive source; The sink a negative source.
From physics we know that
S
E dS
q
0
If there is positive electric charge in the closed surface, the flux will be positive. If the electric charge is negative, the flux will be negative. In a source-free region where there is no charge, the flux through
surface.
The flux only gives the total source in a closed surface, and it cannot describe the distribution 分布of the source. For this reason,
the divergence is required.
We introduce the ratio比率 of the flux of the vector field A at the point through a closed surface to the volume enclosed by that surface, and the limit极限 of this ratio, as the surface area is made to become vanishingly small at the point, is called the divergence of the vector field at that point, denoted by divA, given by
any closed surface becomes zero.
The flux通量 of the vectors through a closed surface can reveal the properties of the sources and how the sources existed within the closed
ex ey ez x y z
Then the grad of scalar field can be denoted as
grad
2. Flux & Divergence
The surface integral面积分 of the vector field A evaluated over a directed surface S is called the flux through the directed surface S, and it is denoted Circulation环量 & Curl旋度
The line integral of a vector field A evaluated along a closed curve is called the circulation of the vector field A around the curve, and it is denoted by , i.e.
A dl
l
If the direction of the vector field A is the same as that of the line
element dl everywhere along the curve, then the circulation > 0. If they are in opposite direction, then < 0 . Hence, the circulation can provide a description of the rotational property of a vector field.
A dS
S
The flux could be positive, negative, or zero.
A source in the closed surface produces a positive integral, while a sink gives rise to a negative one.
l
B dl 0 I
where the flowing direction of the current I and the direction of the
directed curve l adhere to the right hand rule. The circulation is therefore an indication of the intensity of a source. However, the circulation only stands for the total source, and it is unable to describe the distribution of the source. Hence, the rotation is required.
divA lim
ΔV 0
S
A dS ΔV
Where “div” is the observation of the word “divergence, and V is the volume closed by the closed surface. It shows that the divergence of a vector field is a scalar field, and it can be considered as the flux through the surface per unit volume.
From physics, we know that the circulation of the magnetic flux density B around a closed curve l is equal to the product of the conduction current I enclosed by the closed curve and the permeability 磁导率 in free space, i.e.
Curl is a vector. If the curl of the vector field A is denoted by curl A . The direction is that to which the circulation of the vector A will be maximum, while the magnitude of the curl vector is equal to the maximum circulation intensity about its direction, i.e.
Divergence Theorem
