雨流计数法
对“雨流计数法”介绍

对“雨流计数法”介绍雨流计数法(Rainflow counting method)是一种用于疲劳寿命预测和疲劳损伤分析的统计方法。
它通过对载荷历程数据进行处理,识别出载荷的循环次数、振幅和平均值,并统计不同振幅下的循环次数。
雨流计数法广泛应用于许多领域,如机械、航空航天、汽车工程、桥梁工程和风力发电等。
在这篇文章中,将详细介绍雨流计数法的原理、应用和计算过程。
1.雨流计数法的原理和背景疲劳是材料或结构在反复加载下逐渐发生的累积损伤。
在实际工程中,由于载荷的不断变化,对结构的疲劳寿命进行预测和分析是非常重要的。
雨流计数法是一种基于峰谷循环的疲劳分析方法,它通过将载荷历程数据进行循环切分和统计得出结构的振幅、平均值等参数,从而得到结构的疲劳损伤。
2.雨流计数法的应用领域雨流计数法被广泛应用于各个领域,如机械工程、航空航天工程、汽车工程和桥梁工程等。
在机械工程领域,雨流计数法用于预测零件的疲劳寿命,从而指导设计和维护;在航空航天工程中,雨流计数法用于分析航空器部件的疲劳损伤,评估其可靠性和安全性;在汽车工程领域,雨流计数法用于评估引擎和变速器等零部件的疲劳性能;在桥梁工程领域,雨流计数法用于分析桥梁结构的疲劳寿命,指导维护和修复工作。
3.雨流计数法的计算过程雨流计数法的计算过程可以分为循环切分和计数两个步骤。
下面将介绍每个步骤的具体操作。
3.1循环切分循环切分是将载荷历程数据切分成许多不同的循环,即找到载荷历程中的峰谷点。
切分规则如下:(1)初始点:选择载荷历程的起点作为初始点。
(2)峰值点:从初始点开始,寻找下一个大于初始点载荷的点作为峰值点。
(3)谷值点:从峰值点开始,寻找下一个小于峰值点载荷的点作为谷值点。
(4)循环结束点:从谷值点开始,寻找下一个大于谷值点载荷的点作为循环结束点。
(5)将以上得到的峰谷点作为一个循环,将循环次数、振幅和平均值记录下来。
3.2循环计数循环计数是统计不同振幅下的循环次数。
雨流计数法及其载荷谱的编制

雨流计数法及其载荷谱的编制采用雨流计数法完成载荷循环的计数工作,并使该过程可视化,最后实现载荷谱的编制。
标签:雨流计数法;载荷谱;循环1 简化雨流计数法雨流计数法的基本流程,见表一:雨流计数过程模拟图(表一)(1)雨流在实验记录的起点和依次在每一个峰、谷估值的内边开始,亦即从1, 2, 3……等尖点开始。
(2)雨流在流到峰值处(即屋檐)竖直下滴,己知流到对面有一个比开始时最大值(或最小值)更正的最大值(或更负的最小值)为止。
(3)当雨流遇到来自上面的屋顶流下的雨时,就停止流动。
(4)如果初始应变为拉应变,顺序的始点是拉应变最小值的点。
(5)在一雨流的水平长度是作为该应变幅值的半循环计数的。
上述过程可用程序来实现其流程图见表二:表二2 载荷谱的编制过程程序设计流程将程序分为人机界面、输入载荷、雨流计数、输出载荷谱四个模块来实现上述任务。
载荷谱的主要编制方法流程见表三:表三2.1 数据采集程序主要是和ADAM5510以及NI6023E通信正常后,根据采集模块的要求来实现具体采集功能的程序2.2 由于采集数据量较大需要使用数据库来管理这些数据,这就对数据库管理和matlab程序的接口、数据库操作提出了要求,具体来说此模块程序要能够完成以下功能:(1)提供SQLserver2000和matlab连接的接口功能;(2)能够在形成俘获矩阵人工判断数据足够后,matlab程序可以和数据库程序正常链接,能够自动把采集到的程序加入到数据库中去;(3)能够正常查询数据库中的数据,完成基本的数据库数据创建、存取、删除等功能。
2.3 图表显示模块是机械载荷测试系统的结论部分,主要功能在于完成从数据库导出的数据的计算、图表显示、对比显示等功能2.4 人机界面模块是载荷测试系统的外在风格显示,主要包括程序启动和登录界面,上述模块的正确链接,并能够给出错误提示3 结论本文对机械零件的随机载荷进行了研究,分析了雨流计数法这一统计方法,最后采用计算机自动绘制载荷谱,这样大大减轻了疲劳试验的工作量,完成了对疲劳实验数据的自动处理,可以为疲劳寿命预测打下基础。
雨流计数法实例

雨流计数法实例雨流计数法是一种用于疲劳分析的方法,它能够帮助工程师预测材料在持续交变载荷下的寿命。
在现实世界中,许多结构或材料都会受到交变载荷的作用,如果不进行疲劳分析和寿命预测,就会导致结构的失效和事故的发生。
因此,雨流计数法是一个非常重要的工具,它可以帮助工程师评估材料的可靠性和安全性。
雨流计数法的原理是将复杂的交变载荷序列转化为一系列等效的交变载荷块。
通过将载荷序列分解成载荷范围和载荷平均值的组合,可以准确地计算出材料的应力历程。
然后,根据材料的疲劳性能曲线,可以计算出材料在每个载荷块下的寿命。
最后,将所有载荷块的寿命相加,就得到了整个载荷序列下的寿命。
举个例子来说明雨流计数法的应用。
假设我们需要评估一根钢梁在交变载荷下的疲劳寿命。
首先,我们需要获取钢梁所受到的载荷历程数据。
可以通过实验或者数值模拟来获取这些数据。
然后,我们可以将载荷历程数据进行处理,将其转化为一系列的载荷块。
接下来,我们需要使用材料的疲劳性能曲线来计算每个载荷块的寿命。
疲劳性能曲线是一种描述材料在不同应力水平下的寿命的曲线。
可以通过实验或者经验公式来获取这些曲线。
根据疲劳性能曲线,我们可以计算出每个载荷块的寿命。
最后,我们将所有载荷块的寿命相加,就得到了整个载荷序列下的寿命。
通过这种方式,我们可以预测出钢梁在特定载荷下的疲劳寿命。
如果计算出的寿命超过了设计要求,就需要采取相应的措施来增加结构的可靠性,比如增加材料的强度或者改变结构的形状。
除了钢梁,雨流计数法还可以应用于其他许多工程领域。
比如,它可以用于评估桥梁、飞机、汽车等结构在交变载荷下的疲劳寿命。
通过对结构的疲劳寿命进行分析,可以帮助工程师更好地设计和维护这些结构,从而提高结构的安全性和可靠性。
总之,雨流计数法是一种非常重要的工具,可以帮助工程师预测材料在持续交变载荷下的寿命。
它的原理是将复杂的载荷序列转化为一系列等效的载荷块,然后根据材料的疲劳性能曲线计算出每个载荷块的寿命,最后将所有载荷块的寿命相加得到整个载荷序列的寿命。
对“雨流计数法”介绍

雨流计数法简介0、前言机械的疲劳失效是机械失效的主要失效方式,因此对机械失效的主要研究是机械疲劳失效. 目前, 机械疲劳失效的研究有两个方面: 一是根据求出的载荷谱来确定加载程序在试验室或者试验台上对机械进行疲劳试验, 得出机械(材料)在该工况下的实际寿命; 二是根据机械(材料)的特性与载荷谱并且用Miner 准则来估计机械的疲劳寿命. 无论是做疲劳试验还是估计疲劳寿命, 载荷谱的统计都是问题的关键[1]。
1、雨流计数法简介雨流计数法又可称为“塔顶法”,是由英国的Matsuiski和Endo 两位工程师提出的, 距今已有50 多年。
雨流计数法主要用于工程界, 特别在疲劳寿命计算中运用非常广泛。
由来请参看图1, 把应变-时间历程数据记录转过90°,时间坐标轴竖直向下, 数据记录犹如一系列屋面, 雨水顺着屋面往下流, 故称为雨流计数法[2]。
雨流计数法的基本原理[3]如图1所示, 第一个雨流自0点处第一个谷的内侧流下, 从1点落1’后流至5, 然后下落。
第二个雨流从峰1点内侧流至2点落下, 由于1点的峰值低于5点的峰值,故停止。
第三个雨流自谷2点的内侧流到3, 自3点落下至3’ , 流到1’处碰上上面屋顶流下的雨流而停止。
如此下去, 可以得到如下的计数循环块:3-4-3’、1-2-1’、6-7- 6’、8-9- 8’、11-12-11、13-14-13’和12-15-12’。
雨流计数的基本流程如下。
(1) 根据采样定理作数据采集,得到时间历程记录,若截止频率为f c,则采样间隔Δt≤1/ 2f c(2) 根据连续的3个采样数据,删除既不是峰值也不是谷值的数据点,将时间历程记录转化为峰谷值序列。
(3) 针对峰谷值序列采用4点法雨流计数原则进行雨流计数,计数条件如下。
①如果A>B;B≥D;C≤A,记录一个循环 (全波) BCB′,如图 2 所示。
得到范围值S range=|B -C|幅值S a=|B -C|/ 2平均值S m=(B +C)/ 2②如果A <B;B≤D;C≥A,记录一个循环(全波) BCB′,如图 3 所示。
疲劳与断裂2.6 雨流计数法

疲劳与断裂土木工程与力学学院2.6雨流计数法工程中经常需要面对材料或结构在随机载荷作用下的寿命预测问题。
如果能够将随机载荷谱转化为变幅载荷谱,就可以利用变幅载荷谱的寿命预测方法来解决随机载荷谱的寿命预测问题。
将不规则的、随机的载荷-时间历程,转化成为一系列载荷循环的方法,称为循环计数法。
循环计数法有很多种,雨流计数法是常用的一种。
假设随机载荷谱可以看作是以典型载荷谱块为基础重复的载荷-时间历程。
通过雨流计数法,就可以识别出典型载荷谱块所包含的一系列载荷循环,从而可以将其转化为由这一系列载荷循环构成的变幅载荷-时间历程,即变幅载荷谱。
雨流计数法的主要步骤:1)从随机载荷谱中分别选取最大峰或谷处作为典型载荷谱块的起止点,如图1-1'在最大峰处起止,2-2'在最大谷处起止。
雨流计数法的主要步骤:2)将典型载荷谱块重新画在坐标图中,并顺时针旋转90º。
雨流计数法的主要步骤:2)将典型载荷谱块重新画在坐标图中,并顺时针旋转90º。
将载荷-时间历程曲线看作一个多层屋顶,假想有雨滴从最大峰或谷处开始,顺着屋面往下流。
当雨滴流至该层屋面的另一端时,若无下层屋面遮挡,雨滴反向;若有下层屋面遮挡,则雨滴落至下层屋面,并继续顺着该层屋面往下流。
雨流计数法的主要步骤:2)将典型载荷谱块重新画在坐标图中,并顺时针旋转90º。
在图中,雨滴首先从最大峰A处开始,沿屋面AB流动。
到达B点后,有下层屋面CD遮挡,因此落至屋面CD。
接下来,顺着屋面CD流至端点D,因为再无下层屋面遮挡,雨滴反向沿屋面DE流至点E。
又遇下层屋面JA'遮挡,继续下落至屋面JA'。
最后,顺着屋面JA'流至点A'。
至此,该雨滴流过的路径为ABDEA'。
雨流计数法的主要步骤:3)记录雨滴流过的路径,就可以获得一个完整的载荷循环。
载荷循环的主要参量可以从图中获得。
载荷循环ABDEA'或ADA'的应力范围,平均应力。
matlab雨流计数法简单讲解

在工程中,特别是机械结构、航空航天等领域,在对应力、载荷进行疲劳分析时,雨流计数法是一种常用的方法。
这种方法可以有效地对载荷数据进行处理,得到疲劳载荷的循环次数,从而进行疲劳寿命的预测。
1. 什么是雨流计数法?雨流计数法是一种用来对载荷数据进行疲劳分析的方法。
它可以将连续的载荷数据序列转换为一系列载荷循环,然后对这些循环进行统计分析。
通过这种方法,我们可以得到载荷循环的幅值和次数,从而可以进一步进行疲劳寿命的预测和分析。
2. 雨流计数法的基本原理雨流计数法的基本原理是将载荷数据序列分解成一系列循环载荷,然后对这些循环进行统计。
在实际应用中,通常使用峰谷识别法来检测载荷数据中的峰值和谷值,然后根据这些峰值和谷值来进行载荷循环的划分。
可以根据载荷循环的幅值和次数进行统计分析,得到疲劳载荷的循环次数。
3. 雨流计数法的优点和局限性雨流计数法的优点在于可以较为准确地对载荷数据进行处理,得到疲劳载荷的循环次数,从而进行疲劳寿命的预测。
但是,这种方法也存在一定的局限性,例如在处理复杂载荷时,可能会出现较大的误差,需要进行一定的修正和优化。
4. 我对雨流计数法的个人观点和理解在我看来,雨流计数法是一种简单而有效的方法,在工程实践中具有广泛的应用前景。
通过对载荷数据进行分解和统计,我们可以更好地理解载荷对结构疲劳寿命的影响,从而有针对性地进行疲劳设计和分析。
但是,在实际应用中,我们也需要注意方法的局限性,结合具体情况进行合理的选择和修正。
总结回顾:通过本文的讲解,我们了解了雨流计数法的基本原理和应用。
这种方法可以帮助我们对载荷数据进行处理,得到疲劳载荷的循环次数,从而进行疲劳寿命的预测和分析。
在工程实践中,雨流计数法具有重要的意义,但同时也需要注意其局限性,进行合理的修正和优化。
希望通过本文的讲解,读者对雨流计数法有了更深入的了解。
在此,我为您撰写了一篇有关“matlab雨流计数法简单讲解”的文章,希望能够对您有所帮助。
雨流计数法原理

雨流计数法原理
雨流计数法:
1.什么是雨流计数法?
雨流计数法是一种水文学观测方法,主要用来统计河水或雨水流量,帮助研究调查降水、水(或雨)汇、水位、湖泊变化,以及供水、排污、地表面水循环等的变化情况。
2.原理介绍
雨流计数法的原理就是在河道中设置检测站或流量检测回路,将到达检测站的流量折合成静态水位,从而统计测量水位及流量。
通常这种方法是采用雨流量计安装在墙壁上,当流水流过时会被探测器检测,并产生数字信号,随后表达出实际的流量数据,而这些数据会被统计分析,以便理解河道或坑塘洪水的变化状况。
3.应用范围
雨流计数法是应用广泛的水文学观测方法,主要用于:
(1)供水工程中的供水量检测,主要检测能源汇流影响的蓄水工程的
实际取水量;
(2)应急管理中的洪水报警,用于调查水域洪涝灾害的发生情况;
(3)水文勘测中的水量计算,可以辅助水文勘测人员更好地分析水位
变化数据;
(4)水资源综合调查与控制中量化河流水量,确定河流出流控制措施;
(5)水利规划中河流水利设施有效性评价,及河流水利改造方案的研
究调查。
4.优点及局限性
(1)优点:
a.测量速度快,可随时获取实时数据;
b.测量成本较低;
c.流量计量精度高,容旧程度高;
d.测量结果准确可靠,具有较强的客观性。
(2)局限性:
a.对不同流量环境应用有限,只能测量弱流量;
b.对回流复流效应不敏感;
c.为实现多点联网和远程传输,需要更加完善的购置和部署方案。
雨流计数法

疲劳分析中的雨流计数法这种方法的突出特点是根据所研究材料的应力-应变之间的非线性关系来进行计数,亦即把样本记录用雨流法定出一系列闭合的应力-应变滞后环。
参看图1,把应变-时间历程样本记录转过90°,时间坐标轴竖直向下,样本记录犹如一系列屋面,雨水顺着屋面往下流,故称为雨流法。
雨流法有下列规则:(ⅰ)雨流在试验记录的起点和依此在每一个峰值的内边开始,亦即从1,2,3…等尖点开始。
(ⅱ)雨流在流到峰值处(即屋檐)竖直下滴,一直流到对面有一个比开始时最大值(或最小值)更正的最大值(或更负的最小值)为止。
(ⅲ)当雨流遇到来自上面屋顶流下的雨时,就停止流动。
(ⅳ)如果初始应变为拉应变,顺序的始点是拉应变最小值的点。
(ⅴ)每一雨流的水平长度是作为该应变幅值的半循环计数的.在图1中,雨流法从1点开始,该点认为是最小值。
雨流流至2点,竖直下滴到3与4点幅值间的2ˊ点,然后流到4点,最后停于比1点更负的峰值5的对应处。
得出一个从1到4的半循环。
下一个雨流从峰值2点开始,流经3点,停于4点的对面,因为4点是比开始的2点具有更正的最大值,得出一个半循环2-3。
第三个流动从3点开始,因为遇到由2点滴下的雨流,所以终止于2ˊ点,得出半循环3-2ˊ。
这样,3-2和2-3就形成了一个闭合的应力-应变回路环,它们配成一个完全的循环2′-3-2。
下一个雨流从峰值4开始,流经5点,竖直下滴到6和7之间的5ˊ点,继续往下流,再从7点竖直下滴到峰值10的对面,因为10点比4点具有更正的最大值。
得出半循环4-5-7。
第五个流动从5点开始,流到6点,竖直下滴,终止于7点的对面,因为7点比5点具有更负的极小值。
取出半循环5-6。
第六个流动从6点开始,因为遇到由5点滴下的雨滴,所以流到5ˊ点终止。
半循环6-5与5-6配成一个完全循环5ˊ-6-5,取出5ˊ-6-5。
第七个流动从7点开始,经过8点,下落到9-10线上的8ˊ点,然后到最后的峰值10,取出半循环7-8-10。
雨流计数法计算实例

雨流计数法计算实例雨流计数法是一种用于疲劳分析的方法,通过对加载历史进行处理,可以得到疲劳加载的等效循环次数。
在工程实践中,准确估计材料的疲劳寿命是至关重要的,因为过度的疲劳加载可能导致结构的失效。
该方法的基本原理是将复杂的加载历史转化为一系列的循环次数和加载幅值,然后根据统计学方法计算等效循环次数。
这种方法对于不规则的加载历史特别有用,因为它可以准确地估计不同加载幅值下的疲劳寿命。
下面举一个简单的例子来说明如何使用雨流计数法计算疲劳寿命:假设有一个加载历史如下:[10, 15, 20, 8, -5, 12, 6, -2, 18, 10, 5, -4]首先,我们需要将加载历史转化为一系列的循环次数和加载幅值。
对于上述加载历史,我们可以得到以下循环列表:- 循环1:[10, 15, 20, 8]- 循环2:[8, -5, 12, 6, -2]- 循环3:[6, -2, 18, 10]- 循环4:[10, 5, -4]然后,我们根据每个循环的加载幅值和次数来计算等效循环次数。
可以使用下面的公式来计算:等效循环次数 = 循环次数 * (加载幅值 / 平均加载幅值)^b其中,b是一个经验系数,通常取值为0.06到0.10,平均加载幅值是所有循环加载幅值的平均值。
假设b取0.06,平均加载幅值为8.3(通过计算所有循环加载幅值的平均值得到),我们可以得到以下等效循环次数:- 循环1:4 * (8 / 8.3)^0.06 = 4- 循环2:5 * (7 / 8.3)^0.06 ≈ 4.77- 循环3:4 * (8 / 8.3)^0.06 ≈ 3.83- 循环4:3 * (6 / 8.3)^0.06 ≈ 2.56最后,将所有等效循环次数相加,得到总的等效循环次数:总的等效循环次数 = 4 + 4.77 + 3.83 + 2.56 ≈ 15.16通过雨流计数法,我们得到了总的等效循环次数,这个数值可以用来估计材料的疲劳寿命。
雨流计数法的基本原理

雨流计数法的基本原理
2007年07月15日星期日 10:40
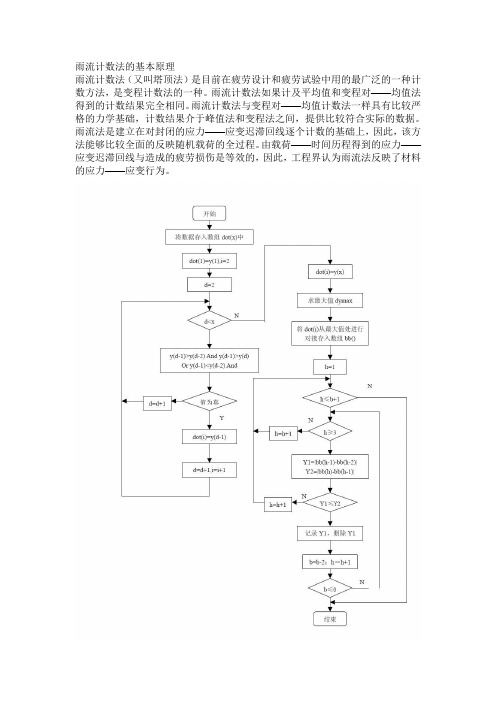
雨流计数法(又叫塔顶法)是目前在疲劳设计和疲劳试验中用的最广泛的一种计数方法,是变程计数法的一种。
雨流计数法如果计及平均值和变程对——均值法得到的计数结果完全相同。
雨流计数法与变程对——均值计数法一样具有比较严格的力学基础,计数结果介于峰值法和变程法之间,提供比较符合实际的数据。
雨流法是建立在对封闭的应力——应变迟滞回线逐个计数的基础上,因此,该方法能够比较全面的反映随机载荷的全过程。
由载荷——时间历程得到的应力——应变迟滞回线与造成的疲劳损伤是等效的,因此,工程界认为雨流法反映了材料的应力——应变行为。
雨流计数法-1
雨流计数法的基本原理
雨流计数法(又叫塔顶法)是目前在疲劳设计和疲劳试验中用的最广泛的一种计数方法,是变程计数法的一种。
雨流计数法如果计及平均值和变程对——均值法得到的计数结果完全相同。
雨流计数法与变程对——均值计数法一样具有比较严格的力学基础,计数结果介于峰值法和变程法之间,提供比较符合实际的数据。
雨流法是建立在对封闭的应力——应变迟滞回线逐个计数的基础上,因此,该方法能够比较全面的反映随机载荷的全过程。
由载荷——时间历程得到的应力——应变迟滞回线与造成的疲劳损伤是等效的,因此,工程界认为雨流法反映了材料的应力——应变行为。
雨流计数法文献综述

雨流计数法0、前言机械的疲劳失效是机械失效的主要失效方式,因此对机械失效的主要研究是机械疲劳失效. 目前, 机械疲劳失效的研究有两个方面: 一是根据求出的载荷谱来确定加载程序在试验室或者试验台上对机械进行疲劳试验, 得出机械(材料)在该工况下的实际寿命; 二是根据机械(材料)的特性与载荷谱并且用Miner 准则来估计机械的疲劳寿命. 无论是做疲劳试验还是估计疲劳寿命, 载荷谱的统计都是问题的关键[1]。
1、雨流计数法简介雨流计数法又可称为“塔顶法”,是由英国的Matsuiski和Endo 两位工程师提出的, 距今已有50 多年。
雨流计数法主要用于工程界, 特别在疲劳寿命计算中运用非常广泛。
由来请参看图1, 把应变-时间历程数据记录转过90°,时间坐标轴竖直向下, 数据记录犹如一系列屋面, 雨水顺着屋面往下流, 故称为雨流计数法[2]。
雨流计数法的基本原理[3]如图1所示, 第一个雨流自0点处第一个谷的内侧流下, 从1点落1’后流至5, 然后下落。
第二个雨流从峰1点内侧流至2点落下, 由于1点的峰值低于5点的峰值,故停止。
第三个雨流自谷2点的内侧流到3, 自3点落下至3’, 流到1’处碰上上面屋顶流下的雨流而停止。
如此下去, 可以得到如下的计数循环块:3-4-3’、1-2-1’、6-7- 6’、8-9- 8’、11-12-11、13-14-13’和12-15-12’。
1.1 雨流计数的基本流程如下。
(1) 根据采样定理作数据采集,得到时间历程记录,若截止频率为 f c,则采样间隔Δt≤1/ 2f c(2) 根据连续的3个采样数据,删除既不是峰值也不是谷值的数据点,将时间历程记录转化为峰谷值序列。
(3) 针对峰谷值序列采用4点法雨流计数原则进行雨流计数,计数条件如下。
① 如果 A>B;B≥D;C≤A,记录一个循环 (全波) BCB′,如图 2 所示。
得到范围值S range=|B -C|幅值S a=|B -C|/ 2平均值S m=(B +C)/ 2② 如果 A <B;B≤D;C≥A,记录一个循环(全波) BCB′,如图 3 所示。
雨流计数法四点法原理

雨流计数法四点法原理雨流计数法是一种用于疲劳寿命评估的方法,它可以对应力历程进行分析,通过统计应力的循环次数和幅值,来估计材料的疲劳寿命。
四点法是雨流计数法中常用的一种方法,它能够对应力历程进行有效的提取和计数。
四点法的原理可以简单地概括为四个步骤:极大值点提取、极小值点提取、交叉点提取和计数。
我们需要从应力历程中提取出所有的极大值点。
极大值点是应力历程中的峰值,可以通过比较相邻点的大小来确定。
例如,如果一个点的值大于它的前后两个点,那么它就是一个极大值点。
接着,我们需要从应力历程中提取出所有的极小值点。
极小值点是应力历程中的谷底,同样可以通过比较相邻点的大小来确定。
例如,如果一个点的值小于它的前后两个点,那么它就是一个极小值点。
通过极大值点和极小值点,我们可以确定出应力历程中的波峰和波谷。
接下来,我们需要找到波峰和波谷之间的交叉点。
交叉点是波峰和波谷之间的过渡点,可以通过比较相邻点的大小来确定。
例如,如果一个点的值大于前一个点并且小于后一个点,那么它就是一个交叉点。
我们需要对交叉点进行计数。
计数的目的是统计应力历程中的循环次数和幅值。
对于每一个交叉点,我们都可以得到一个循环的幅值,即波峰与波谷之间的差值。
通过统计所有的循环幅值,我们可以得到应力历程的循环次数和幅值的分布情况。
通过四点法的原理,我们可以对应力历程进行有效的提取和计数,从而得到疲劳寿命的估计。
这种方法可以应用于各种材料和结构的疲劳分析,帮助我们评估材料的使用寿命和可靠性。
总结起来,雨流计数法四点法是一种用于疲劳寿命评估的方法,通过四个步骤对应力历程进行提取和计数,得到循环次数和幅值的分布情况。
这种方法在工程实践中具有广泛的应用价值,可以帮助我们预测材料的疲劳寿命,优化设计和维护策略,提高产品的可靠性和安全性。
雨流计数法计算实例

雨流计数法计算实例雨流计数法(Rainflow Counting Method)是一种用于计算疲劳载荷的方法,适用于结构、材料等领域的疲劳寿命预测。
它可以将复杂的载荷历程转化为等效疲劳载荷循环,从而进行疲劳寿命的估算。
雨流计数法的基本原理是将载荷历程分解为一系列互不重叠的载荷循环,然后统计每个循环的幅值和次数。
主要的步骤包括载荷振幅范围的计算、载荷循环的识别和载荷循环的计数。
首先,对于给定的载荷历程,需要先进行滤波处理,去除高频成分。
然后将载荷历程进行幅值统计,即计算每个采样点的载荷振幅。
这里需要注意的是,振幅的计算通常选取主方向的振幅,即载荷历程在主方向上的振幅。
接下来是载荷循环的识别,这一步是找到载荷历程中的正循环和负循环。
正循环是载荷由最小值变为最大值再返回最小值的部分,负循环则相反。
一个完整的载荷循环包含了一个正循环和一个负循环。
为了准确识别载荷循环,通常采用峰谷顶点法或升序降序法。
峰谷顶点法是通过找出载荷历程的峰值和谷值,并将它们连线,然后通过顶点来确定循环的开始和结束点。
顶点是指一个峰或一个谷与其相邻的两个谷或峰之间的交点。
升序降序法则是通过找出历程中的最大值和最小值,然后确定其出现的顺序来识别循环。
最后是进行载荷循环的计数。
计数的原则是:当载荷循环的幅值和次数已经记录过时,相同的循环不再计数。
这一步可以利用一个循环计数矩阵来实现。
根据循环的幅值和次数,循环计数矩阵可以记录下所有不重复的循环。
除了基本的步骤外,还有一些改进的雨流计数法方法,如修正的雨流计数法、多重指标的雨流计数法等。
修正的雨流计数法在传统的雨流计数法基础上考虑了载荷历程的非线性特性,能更准确地估算疲劳寿命。
多重指标的雨流计数法则是引入了多个载荷指标,以更好地描述载荷循环。
总而言之,雨流计数法是一种有效的计算疲劳载荷的方法,通过将复杂的载荷历程转化为等效的载荷循环实现疲劳寿命预测。
其基本步骤包括滤波处理、载荷振幅的计算、载荷循环的识别和计数。
雨流计数法python程序-概述说明以及解释

雨流计数法python程序-概述说明以及解释1.引言1.1 概述雨流计数法是一种用于疲劳寿命预测和分析的有效方法,通过对加载历史数据进行循环计数和幅值计算,可以较准确地评估结构在长期循环加载下的疲劳性能。
本文将介绍如何使用Python语言实现雨流计数法,以及通过编写程序实现对加载数据的分析。
在本文中,我们将首先介绍雨流计数法的基本原理和步骤,以及其在工程实践中的应用。
接着,我们将详细讲解如何使用Python编写雨流计数法的程序,包括加载历史数据、循环计数和幅值计算等关键步骤。
最后,我们将通过示例程序和结果分析展示程序的运行效果,以及如何利用计算得到的结果进行疲劳寿命预测。
通过本文的阅读和实践,读者将了解雨流计数法的实现原理和方法,以及如何通过编写Python程序实现对加载数据的有效分析。
同时,本文也将对雨流计数法在工程实践中的应用进行总结和展望,为读者提供更深入的思考和研究方向。
1.2 文章结构:本文主要分为引言、正文和结论三个部分。
其中引言部分将介绍雨流计数法的概念和重要性,以及文章的目的和结构安排。
正文部分将详细介绍雨流计数法的概述和原理,以及通过Python实现雨流计数法的方法。
最后,结合示例程序和结果分析,展示雨流计数法在实际工程中的应用和效果。
结论部分将对整篇文章进行总结,探讨雨流计数法的应用价值,并展望其在未来可能的发展方向。
整个文章结构严谨,内容丰富,旨在为读者提供全面的雨流计数法Python程序知识。
1.3 目的本文的主要目的是介绍如何使用Python编程语言实现雨流计数法。
雨流计数法是一种用于疲劳寿命预测和结构健康监测的重要方法。
通过本文的学习,读者可以了解到雨流计数法的基本原理和实现步骤,掌握如何利用Python编写程序进行雨流计数的计算。
在实际工程应用中,雨流计数法可以帮助工程师准确评估结构的疲劳寿命,预测可能出现的疲劳破坏情况,从而及时采取必要的维护和修复措施,保障结构的安全运行。
雨流计数法excel

雨流计数法excel雨流计数法(Rainflow Counting Method)是一种用于疲劳寿命预测和疲劳分析的方法。
它的应用范围广泛,可以用于工程结构、材料研究、机械设备等领域。
在这篇文章中,我们将详细介绍雨流计数法在Excel中的应用。
雨流计数法的基本原理是将应力或应变时间历程分解为一系列循环载荷,然后对这些循环进行计数和分析。
它的核心思想是将复杂的应力或应变历程简化为一系列疲劳循环,从而减少分析的复杂度。
在Excel中,我们可以利用一些函数和工具来实现雨流计数法。
首先,我们需要将应力或应变时间历程数据导入Excel表格中。
可以使用Excel的数据导入功能,或者手动输入数据。
确保数据准确无误后,我们可以开始进行雨流计数的操作。
雨流计数的第一步是将原始数据进行平滑处理,以去除噪声和杂波。
可以使用Excel的平滑函数或滤波器来实现这一步骤。
平滑后的数据更易于分析和计数。
接下来,我们需要确定循环的起点和终点。
循环的起点是一个应力或应变极值点,而终点是下一个应力或应变极值点。
在Excel中,我们可以使用MAX和MIN函数来找到极值点,并使用IF函数来确定起点和终点。
一旦确定了循环的起点和终点,我们可以使用一个循环计数器来计数循环的个数。
可以使用Excel的循环函数或宏来实现这一功能。
计数器将循环的起点和终点相连,并自动计数。
计数完毕后,我们可以得到一系列疲劳循环的振幅和次数。
振幅表示循环的幅值大小,次数表示该幅值出现的次数。
可以使用Excel 的排序和计数函数来对振幅进行排序和统计。
我们可以根据振幅和次数绘制疲劳循环图。
可以使用Excel的图表功能来绘制柱状图或曲线图。
图表可以直观地展示不同振幅下循环的次数,帮助我们分析疲劳寿命和疲劳强度。
除了基本的雨流计数法,我们还可以在Excel中进行一些扩展和改进。
例如,可以结合统计分析和概率分布函数,对疲劳循环进行更精确的预测。
可以使用Excel的统计函数和概率分布函数来实现这一功能。
ncode雨流计数法导出数据

ncode雨流计数法导出数据ncode雨流计数法是一种用于疲劳寿命预测和结构可靠性评估的方法。
本文将从标题为导出数据的角度,介绍该方法的原理和应用。
一、什么是ncode雨流计数法?ncode雨流计数法是一种疲劳寿命预测方法,它通过将结构的应力历程转化为等效应力循环数据,进而预测结构在实际工况下的疲劳寿命。
所谓的“雨流计数”是指将应力历程中的应力循环分解为一个个雨流循环,并统计其循环次数,从而确定等效应力循环。
二、数据导出的过程1. 数据采集在进行ncode雨流计数法之前,首先需要采集结构的应力历程数据。
这可以通过传感器等装置实时监测结构的应力变化,并将数据记录下来。
2. 数据处理通过采集到的应力历程数据,我们可以利用ncode雨流计数法将其转化为等效应力循环数据。
具体的数据处理过程包括以下步骤:(1) 雨流循环的提取:将应力历程中的应力峰谷点找出,并以这些点为基础,将应力历程分解为一个个雨流循环。
(2) 循环次数的统计:对每个雨流循环进行统计,记录其循环次数。
(3) 等效应力的计算:根据每个雨流循环的应力幅值和循环次数,计算得到等效应力。
3. 导出数据经过以上的数据处理步骤,我们可以得到每个雨流循环的等效应力数据。
这些数据可以导出为表格或文本形式,以便后续的疲劳寿命预测和结构可靠性评估。
三、ncode雨流计数法的应用ncode雨流计数法在工程实践中有着广泛的应用。
它可以帮助工程师预测结构在实际工况下的疲劳寿命,评估结构的可靠性,并优化结构设计。
具体的应用包括以下几个方面:1. 疲劳寿命评估通过利用ncode雨流计数法提取的等效应力循环数据,结合材料的疲劳性能参数,可以对结构在实际工况下的疲劳寿命进行评估。
这对于确保结构的安全运行和延长其使用寿命具有重要意义。
2. 结构可靠性评估基于ncode雨流计数法提取的等效应力循环数据,可以通过可靠性分析方法,对结构在疲劳载荷下的可靠性进行评估。
这有助于工程师了解结构在使用过程中可能出现的疲劳失效风险,并采取相应的措施进行风险控制。
雨流计数法的应用

首先应明确有限元模型对几何模型进行了离散化。这就使几何模型与有限元模型产生了差异。
过盈配合所致的接触分析的难点在于如何确定初始接触状态。初始接触状态设置得不对,会导致错误的计算结果或者不准确的计算结果
因此若想通过几何来模拟过盈配合,应该尽量细化接触面的网格,但同时也增加了计算量。
速度快模态分析单元载荷(压力、温度、加速度等)可用于瞬态分析得到更平滑、精确的响应曲线允许考虑模态阻尼
比完全法快
缺点
计算费用高
恒定时间步长不能计算非线性,只能包含简单的点点接触不能施加强制非零位移
需要扩展得到完整结果不能施加单元载荷所有载荷必须施加在主自由度上时间步长恒定只支持简单的点点接触
完全法求解瞬态动力学的问题
2
3
1
问题和工作
问题
应用何种方法(完全法、模态叠加法)
时间步长的确定,哪些是关心频率(Δt=1/20f)
模型(装配体、整体分析)
工作
简化模型去掉或等效接触
减少单元数量
设置合适的时间步长
比较X和Y的大小。若X<Y则返回(2);若X≥Y,则进行(5).
将Y记做一个循环,删除与Y相应的峰谷值,返回(3)。
ANSYS中过盈配合的模拟
有限元中过盈配合的模拟主要有三种方法:通过几何模拟,应用温度法模拟,通过接触设置模拟。
01
02
03
温度法通过给零件施加不同的温度,产生“热胀冷缩”的效应来模拟过盈。
缩减法。通过主自由度及缩减矩阵压缩问题规模。在主自由度的位移被计算出来后,可将解扩展到完整自由度集上。
03
模态叠加法。通过对模态分析得到的振型乘上一定的因子然后求和来计算结构响应。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
3Rainflow Cycle CountingJ Problem Description 62J Set Up the Fatigue Analysis 63J Run the Fatigue Analysis 69J Review the Results 72J Concluding Remarks 78MSC Fatigue 2005 QuickStart Guide62Problem DescriptionProblem DescriptionThis example is an extension of the previous example where the simple constant amplitude loading isreplaced with a more complex randomly varying time signal.Invoke Pre&Post or MSC Patran by typing the following symbols at the system prompt or from a DOSwindow:fXX or fatX or fatigue where XX is the version numberp3or patranIf you have not already, open the same database that you created in the previous example working in thesame directory from the File | Open menu. The name of the database should be keyhole.Objective•To predict the life of the keyhole subject to a varying load signal.•To understand how to normalize the FE stresses.•To introduce the concept to rainflow cycle counting.•To introduce the concept of damage summation.•To investigate the effect of mean stress.•To investigate the probabilistic nature of fatigue.Note:The geometry and materials information are identical to that of the previous exercise.Chapter3: Rainflow Cycle Counting63Set Up the Fatigue Analysis Set Up the Fatigue AnalysisTo begin setup for a fatigue analysis press the Analysis switch in Pre&Post (or from the Tools pulldown menu in MSC Patran, select MSC Fatigue and then Main Interface). This will bring up the MSC Fatigue main form from which all parameters, loading and materials information, and analysis control are accessed.Load the Previous S-N Analysis ParametersMSC Fatigue 2005 QuickStart Guide Set Up the Fatigue Analysis 64Instead of defining all the analysis parameters again, let us begin from the last analysis. Once the form is open, type the jobname of the previous example in the Jobname databox (simple_sn ) and issue a carriage return (Return or Enter). You will be prompted to read in an old analysis setup file (it detects a file called simple_sn.fin in your local directory and reads in the parameters).Now change the jobname and the title:1.Jobname: rf_cycle2.Title: Simple S-N Analysis, Variable LoadingLoading InformationOpen the Loading Info... form. Then press the Time History Manager button. This will launch PTIME. The time variation of the load will be defined by a signal called SAETRN which is stored in the loading central database in the MSC Fatigue installation directory.Hint:You can do the same thing in the Job Control... form with the Action set to Read SavedJob.Chapter3: Rainflow Cycle Counting65Set Up the Fatigue Analysis Copy SAETRN from the Central DatabaseWhen PTIME comes up, select Add an entry... and then Copy from central as the method of input. A form will appear that will ask for a name. Use the List button to select SAETRN from the central database.Scale the Time History LoadFrom the PTIME main menu, select Change an entry... and then Polynomial transform. We are goingto scale up the time history to represent the actual loading applied to the component. You will be asked for the Database Entry to transform and a new target file. Use the same name (SAETRN) for both and allow overwrite. The transformation from will then appear. We simply want to scale the load up so all that is needed is to input a scale factor of 10 in the second databox. Press OK when done.Finally a form appears allowing you to change any details associated with this time history. Enter the following:MSC Fatigue 2005 QuickStart Guide66Set Up the Fatigue Analysis1.Description 1: Leave as is2.Description 2: Blank this out3.Load type: Force4.Units: Newtons5.Number of fatigue equivalent units: 16.Fatigue equivalent units: Repeats7.Life results will be reported as the number of Repeats of this entire loading sequence and not asindividual stress cycles as in the previous exercise.Plot the Time HistoryPTIME returns to its main menu where you can select Plot an entry. Accept the default file, SAETRN.Note that the maximum value is close to 10,000 Newtons. As a comparison to the previous example,which oscillated in a fully reversed fashion between positive 10kN and negative 10kN, this signal variessignificantly with a very positive mean and only occasionally reaches or nears the 10kN maximum. Wetherefore would expect this loading to be less damaging with all else the same.Select File | Exit to close the plot and press or double click the eXit switch in PTIME.67Chapter 3: Rainflow Cycle Counting Set Up the Fatigue AnalysisAssociate the FE Load to its Time VariationNow back on the Loading Info... form you must associate the time variation of the load that you just created to the static FE load case. Go to the spreadsheet as was done in the previous example. Two things need to be changed on this form.1.Time History: SAETRNSelect the middle cell to make it active. Another spreadsheet (now with two rows) appears at the bottom of the form from which you select the time history file. Click on the SAETRN row anywhere with the mouse. This will replace the cell with the new time history file name.2.Load Magnitude: 10,000The next cell becomes active and a databox appears below the spreadsheet. Change this entry to 10,000. You must press a carriage return (Return or Enter) to accept the value in the databox and fill the cell in the spreadsheet. Forgetting to do this is a common error.The time variation of the loading is now associated to the static FE results. Press the OK button to close the Loading Info... form.The load magnitude acts as a divisor to normalize the stresses to obtain a stress distribution due to a unit load as in the equation σij (t)=P(t)σij /P fea , where σij and P fea are the stress tensor and load magnitude from the FE analysis, P(t) is the externally defined time variation of the loading, and σij (t) is the resulting timeNote:In the previous example we entered unity for the Load Magnitude accepting the FE load asbeing the true representation of the load and thus the stresses. The time history, UNITLOAD, scaled the stress distribution between 1 and -1 to signify the time variation of the loading. This time the time history SAETRN is used to define the actual loading as it changes with time. The FE load magnitude is therefore simply an arbitrary number used to obtain the stressdistribution. The stresses in the FE analysis need to be normalized by this FE load magnitude of 10kN, to simulate the stress distribution due to a unit load.MSC Fatigue 2005 QuickStart Guide68Set Up the Fatigue Analysisvariation of the stress tensor (at any particular location in the component). This can be done because theanalysis is linear elastic. Using linear elastic FE analysis and associating an external time variation of theloading for fatigue analysis is called the “pseudo-static” method. “It might be said that all stress analysesare basically fatigue analyses, the differences lying in the number of cycles of applied stress.” - quotefrom Carl C. Osgood, Fatigue Design (1982).Chapter3: Rainflow Cycle Counting69Run the Fatigue Analysis Run the Fatigue AnalysisYou are ready to run the fatigue analysis. Open the Job Control... form, set the Action to Full Analysis and press the Apply button. The database will close momentarily as the results information is extracted. When the database reopens, the job will have been submitted. You can then set the Action to Monitor Job and press the Apply button from time to time to view the progress. When the messageFatigue analysis completed successfullyappears, the analysis is complete. Close down the Job Control... form when done.Rainflow Cycle CountingThis analysis takes a few minutes to run to completion. The reason it takes longer than the previous example is due to the complex nature of the time signal. The program is performing a procedure called rainflow cycle counting, referred to as “preprocessing” in MSC Fatigue. Cycle counting is a mechanismto extract and count the number of stress cycles in a signal.MSC Fatigue 2005 QuickStart Guide70Run the Fatigue AnalysisThe term Rainflow is attributed to two Japanese gentlemen, Matsuishi and Endo, who invented themethod. It is based on the concept of rain drops flowing off Japanese style pagoda roofs. Time historysignals are stood on end and rain is visualized to run off of each peak or valley. Various rules wereadopted to count cycles and reversals which is beyond the scope of this text; but suffice it to say that theend result of rainflow cycle counting is a set of constant amplitude signals and a count of the number ofcycles in each. Cycle counts can be visualized as probability density functions (PDF) or as 3-dimensionalhistogram matrices as you will see later.Damage SummationIt is important to break up a variable signal into a number of constant amplitude signals in order to assessthe life from the S-N curve. The curve itself is created by a series of constant amplitude tests. So for eachcycle in the signal you must look up the proper stress from the S-N curve. What stress to look up is thejob of rainflow cycle counting. The next challenge to tackle is the summation of the damage from eachcycle in order to report a total life due to all cycles. This is accomplished by way of the Palmgren-Minerlinear damage summation law.This states that damage can be summed by determining the ratio of the number of cycles experienced tothe number of cycles to failure for a given stress range or level and then summing all the ratios for everystress range. When this number, known as Miner’s Constant, reaches unity, failure is said to haveoccurred. The predicted life is then determined by summing the percentage of life used by each stresslevel for the entire time signal. Life is then reported back as to the number of times the given time signalcan be applied before failure.71Chapter 3: Rainflow Cycle Counting Run the Fatigue AnalysisSpeeding up the AnalysisThere are two ways that you could speed up this analysis.1.First, since we already know where the failure location will be (at the point of highest stress) because of the simplicity of this model, we could have defined a Group with only this node (Node1) and specified it in the Materials Info... form. This however, would only calculate life at this one node and would ignore the rest of the model.2.Second, on the Job Control... form you can turn on the Simplified Analysis toggle. As an exercise after you finish this problem, turn this toggle ON , change the Jobname to something else and re-run the problem. Note how much faster the analysis proceeds relative to the first time. What is happening is that for a normal analysis, the rainflow procedure is being applied to each location once its stress time variation is determined. When the Simplified Analysis toggle is turned ON, the rainflow procedure is applied to the loading time history first and the FE stresses are used to scale the rainflow histogram matrix. This speeds up the analysis significantly for a complex time signal for a single load. It does however, produce slightly less accurate results. Notice the slightvariation in predicted life when you do this.Hint:This is where user-defined fatigue equivalent units come in handy, because rarely does onewant life reported in “repeats” of the time signal, but rather in more meaningful units suchas hours, miles, years, laps, missions, etc. This is accomplished by defining these user-defined units in the PTIME, loading database manager, utility. Use the Change an entry | Edit details option.MSC Fatigue 2005 QuickStart Guide72Review the ResultsReview the ResultsOpen the Results... form on the main MSC Fatigue setup form (not to be confused with the Resultsapplication switch on the main Pre&Post or MSC Patran form). With the Action set to Read Results,press Apply. The fatigue analysis results have been read into the database. You can review the lifecontour plot as you did in the previous exercise if you wish. The contour will look similar but themagnitudes will be different.Tabular ListingOn the MSC Fatigue Results... form, change the Action to List Results and press Apply. This will startthe module PFPOST which tabularly lists the fatigue analysis results. Accepting the jobname and thedefault filtering values by pressing OK a couple of times will get you to the main menu. Press or doubleclick the Most damaged nodes switch to view a tabular listing. Note the life value of approximately105.26=184,000 repeats of the signal on Node 1. This is significantly less damaging than the previousexample considering the life is reported in repeats of the time history and not as individual cycles. To getthe number of cycles, we would have to multiply the life result by the rainflow cycle count. Press Cancelto quit the listing and press or double click eXit to leave PFPOST.Chapter3: Rainflow Cycle Counting73Review the Results Histogram MatrixLet us take a look at the results of a rainflow cycle count. From the Results... form, change the Action to Optimize and press Apply (you do not need to enter a node number) on the Results... form. This will launch the module FEFAT in its design optimization mode. When it comes up, press Worst Case to automatically select the node with the lowest life prediction. Enter a Design Life of 1E6 (a million) repeats. Press the OK button. The analyzer will re-analyze the fatigue life at Node 1 and will report the life value to you. Pressing the End button will put you into the main optimization menu.Select results Display and then plot Cycles histogram. This will display a histogram plot showing the results of the rainflow cycle count for the critical location on the model. It looks a little bit like a city skyline. Note that there are quite a few cycles that have low stress ranges and that there are fewer with high stress ranges. The height of each tower represents the number of cycles at that particular stress range and mean. Each tower is used to look up damage on the S-N curve and damage is summed over all towers.A histogram cycle plot from our first example would yield only a single tower of unit height with a meanof zero.MSC Fatigue 2005 QuickStart Guide Review the Results 74Now convert the cycle histogram plot to a damage histogram plot. This is done by either returning to the main menu and selecting results Display | plot Damage histogram or with the cycle histogram plot still displayed, select Plot_type | Damage . Now you can see the damage caused by each bin. Notice that the lower stress ranges produced zero damage. All damage came from cycles in the higher stress range, which is to be expected. Select File | Exitwhen done viewing the graphics.Hint:The accuracy of the fatigue calculation is dependent on the number of towers allowed in therainflow histogram. Typically it is broken up into what are called bins which is the matrixsize. These bins can be 32x32, 64x64, or 128x128. If you want to increase the accuracy, youcan run FEFAT interactively at the critical location and specify a larger bin size.75Chapter 3: Rainflow Cycle Counting Review the ResultsEffect of Mean StressNow let us investigate the effect of mean stress on the fatigue life predictions. First remember that the S-N curve we are using was produced for an R-ratio of minus one, or no mean stress in other words. The time history used in this example has a predominately tensile mean. The initial life prediction did not take into consideration this mean stress and therefore could perhaps be giving a somewhat non-conservative answer. From FEFAT’s design optimization menu, select Sensitivity analysis | Mean stress correction (all) then press or double click the Recalculate switch. A listing showing no correction plus two mean stress correction methods appear: Goodman and Gerber. Note that both of them give more conservative answers.How is mean stress compensated for in the S-N analysis?The simple way to explain this is that for both the Goodman and Gerber methods, knowing the ultimate tensile strength (S u ) and the actual stress amplitude (σa ) and mean (σm ), an equivalent stress range with zero mean is determined. Goodman and Gerber follow these equations:σa S e -----σm S u------+ 1 Goodman =σa S e -----σm S u ------⎝⎠⎛⎞2+1 Gerber =MSC Fatigue 2005 QuickStart Guide Review the Results 76Graphically this looks like the plot to the right where, at least for Goodman, if you draw a line connecting S u to the intersection of σa and σm and then continue it on to the stress amplitude axis, this will indicate the equivalent stress S e with zero mean. This stress is then used to look up damage on the S-N curve.Probabilistic Nature of FatigueAs a final exercise in this example, let us investigate two different materials as we did in the first problem. From the main menu of FEFAT’s design optimization mode, select Material optimization . Change the material S-N curve from MANTEN_MSN to RQC100_MSN and then press or double click theRecalculate switch again. Note that RQC100_MSN , being a much higher strength steel, gives a much higher life prediction (357,000 repeats vs. 184,000 repeats) for no mean stress corrections. This means RQC100_MSN is a better material to use (or does it?). Just looking at the S-N curve might indicate this also.Press or double click the Original parameters button to put the material back to MANTEN_MSN and then press or double click the Change parameters switch and change the Design Criterion to 99. Press or double click the Recalculate switch. Note the life of approximately 85,400 repeats. Now change the material to RQC100_MSN as done earlier and press or double click the Recalculate switch. The lifeusing the higher strength steel is now only about 30,900 repeats, less than that of the lower strength steel.Note:As a stress range of a cycle becomes larger and larger, there tends to be less and less possiblevariability in the mean of that cycle. This is indicated on the cycle histogram plot since thebase of these type of plots tends to be triangular in nature, which means that as the stress gets larger, the mean stress has less of an effect on the fatigue life.Chapter3: Rainflow Cycle Counting77Review the ResultsThis is due to the probabilistic nature of fatigue and the scatter associated with the S-N curves themselves. By specifying 99 as the design criterion, we are asking MSC Fatigue to calculate a life value based on a 99% certainty of survival. The larger the scatter in the original S-N data that makes up the curve, the less certain we will be of survival and the code takes this into account by reporting a more conservative answer. The default is a 50% probability of survival (or failure)Note:Scatter is associated with S-N curves and other damage curves due to the fact that, for example, if you take 10 identical test coupons and subject them to what you think are identicaltests, you will get ten slightly different answers. The material parameters associated with S-Ncurves take this into consideration with the Standard Error of Log(N) (SE) determined byregression analysis of the raw data.MSC Fatigue 2005 QuickStart Guide78Concluding RemarksConcluding RemarksThis exercise introduced you to rainflow cycle counting, damage summation, mean stress effects, andthe probabilistic nature of fatigue by using a randomly varying load on our simple keyhole model.Though this example still did not help us identify critical locations since we already knew where failurewould occur, it did start to show the power of MSC Fatigue by being able to handle complex time signalsand to make compensation for parameters that may effect the fatigue life, something that would be adaunting task to do by hand.The next exercise will introduce the concept of a component S-N curve.Quit from Pre&Post or MSC Patran when you are through with this exerciseNote:MSC Fatigue does not take into account the frequency (speed at which cycles are experienced) or the sequence (when a particular cycle is experienced relative to other cycles) of cycles froma given signal. Rainflow cycle counting simply counts the number of cycles and determinestheir range and mean. Frequency and sequence can have an influence on the fatigue life but isa third or fourth order effect on life prediction in most cases. MSC Fatigue does provide youwith certain fatigue analysis utilities to determine if these influences are important after theinitial analysis using the MSC MSC Fatigue module MTCD (for time correlated damage).。
