期权期货考试大题
云南省2024年期货从业资格:期权交易的基本策略考试题

云南省2024年期货从业资格:期权交易的基本策略考试题一、单项选择题(共25题,每题2分。
每题的备选项中,只有一个最符合题意)1、甲期货公司共有l0家营业部,现有净资产5000万元,资产调整值为100万元,负债调整值为200万元,有两家客户须要追加保证金,但经催告后仍旧未足额追加,未足额追加的保证金数额达到1000万元,该期货公司客户权益总额为10亿元。
依据以上数据,回答下列问题:甲期货公司的净资本是()。
A.4100万元B.5000万元C.3900万元D.4000万元2、某企业托付期货公司为其办理期货交易。
该企业在某交易日后保证金不足,且未在期货公司规定的时间内刚好追加保证金,也没有自行平仓。
期货公司在未征求该企业看法的状况下将其合约强行平仓,发生费用为X,同时产生损失Y,则下列说法正确的是()。
A.企业担当损失Y,期货公司担当费用XB.期货公司担当费用X和损失YC.企业担当费用X,期货公司担当损失YD.企业担当费用X和损失Y3、期货公司首席风险官的工作底稿和工作记录应当至少保存()年。
A.20B.15C.10D.54、期货从业人员发觉投资者有违法违规的行为时,应()。
A.刚好向主管部门报告B.公允对待该投资者C.刚好向所在期货经营机构报告,留意防范投资者的信用风险D.了解投资者的投资目标5、自然人投资者甲向期货公司会员乙申请开立股指期货交易编码,乙对甲进行了审查,发觉甲有若干地方不太符合标准,于是期货公司会员乙适当调整了一下对投资者的适当性标准,给甲开立了股指期货交易编码。
[依据上述资料,请回答以下问题。
]有权对投资者的适当性标准进行调整的机关是__。
A.期货公司会员B.期货业协会C.期货交易所D.证监会6、下列关于实行全员结算制度的期货交易所的说法,不正确的是______。
A.会员均具有与期货交易所进行结算的资格B.会员由期货公司会员组成C.期货交易所对会员结算D.会员对其受托的客户结算7、《期货从业人员执业行为准则(试行)》自()起施行。
期权期货-复习题

复习题一、单项选择题(在每题给出的4个选项中,只有1项最符合题目要求,请将正确选项的代码填入括号内)1.金融期权合约是一种权利交易的合约,其价格( C )。
A.是期权合约规定的买进或卖出标的资产的价格B.是期权合约标的资产的理论价格C.是期权的买方为获得期权合约所赋予的权利而需支付的费用D.被称为协定价格【解析】金融期权是一种权利的交易。
在期权交易中,期权的买方为获得期权合约所赋予的权利而向期权的卖方支付的费用就是期权的价格。
2.标的物现价为179.50,权利金为3.75、执行价格为177.50的看涨期叔的时间价值为( B )。
A.2B.1.75C.3.75D.5.75【解析】期权的价格即权利金是由两部分构成,一部分是内在价值(立即执行所带来的价值和0取最大),一部分是时间价值,本题内在价值为2,时间价值为3.75-2=1.753.买进执行价格为1200元/吨的小麦期货买权时,期货价格为1190元/吨,若权利金为2元/吨,则这2元/吨为( B )。
A.内涵价值B.时间价值C.内在价值+时间价值D.有效价值【解析】虚值期权无内涵价值,只有时间价值。
4.下列说法错误的是( B )。
A.对于看涨期权来说,现行市价高于执行价格时称期权处于实值状态B.对于看跌期权来说,执行价格低于现行市价时称期权处于实值状态C.期权处于实值状态才可能被执行D.期权的内在价值状态是变化的【解析】对于看跌期权资产现行市价低于执行价格时称为期权处于“实值状态”。
由于标的资产的价格是随时间变化的,所以内在价值也是变化的。
5.期权价值是指期权的现值,不同于期权的到期日价值,下列影响期权价值的因素表述正确的是( A )。
A.股价波动率越高,期权价值越大B.股票价格越高,期权价值越大C.执行价格越高,期权价值越大D.无风险利率越高,期权价值越大【解析】 B、C、D三项都要分是看涨期权还是看跌期权,不能笼统而论。
6.有一项欧式看涨期权,标的股票的当前市价为20元,执行价格为20元,到期日为1年后的同一天,期权价格为2元,若到期日股票市价为23元,则下列计算错误的是( D )。
期货与期权试题

一、单项选择题1.第一家推出期权交易的交易所是C )。
2.期权合约的到期日一般是在( B )到期。
A.期货合约进入交割月之前2个月B.期货合约进入交割月之前1个月C.期货合约进入交割月之后D.期货合约进入最后交易日3.某投资者拥有敲定价格为840美分/浦式耳的3月大豆看涨期权,最新的3月大豆成交价格为美分,浦式耳,那么该投资者拥有的期权属于( C )。
A.实值期权B.深实值期权C.虚值期权D.深虚值期权4.当期权处于( C )状态时,其时间价值最大。
A.实值期权B.虚值期权C.平值期权D.深实值期权5.期权的时间价值随着期权到期日的临近而( D )。
A.增加B.不变C.随机波动D.递减6.在期权交易中,保证金交纳应当( A )。
A.卖方交纳B.卖方交纳C.买卖双方均需要交纳D.买卖双方均不需交纳7.买入看涨期权的风险和收益关系是( A )。
A.损失有限,收益无限B.损失有限,受益有限C.损失无限,收益无限D.损失无限,收益有限8.买入看跌期权的风险和收益关系是B )。
A.损失有限,收益无限B.损失有限,受益有限C.损失无限。
收益无限D.损失无限,收益有限9.卖出看涨期权的风险和收益关系是( D )。
A.损失有限,收益无限B.损失有限,收益有限C.损失无限,收益无限D.损失无限,收益有限10.卖出看跌期权的风险和收益关系是(B )。
A.损失有限,收益无限B.损失有限,受益有限C.损失无限,收益无限D.损失无限,收益有限11.中国某大豆进口商,在5月份即将从美国进口大豆,为了防止价格上涨,2月10日该进口商在CBOT买入40手敲定价格为660美分,浦式耳,5月大豆的看涨期权,权力金为10美分,当时CBOT5月大豆的期货价格为640美分。
当期货价格涨到( B )时,该进口商达到盈亏平衡点。
12.就看涨期权而言,当期权标的物的价格( B )等于期权的执行价格时,内涵价值为零。
A.小于B.大于或等于C.只有大于D.只有等于13.买入跨式期权组合和卖出跨式期权组合的最大区别在于( C )。
期权从业考试题及答案

期权从业考试题及答案一、单选题1. 期权是一种()。
A. 期货合约B. 股票C. 金融衍生品D. 债券答案:C2. 期权的买方拥有的权利是()。
A. 强制卖方履行合约B. 强制买方履行合约C. 选择是否履行合约D. 无条件履行合约答案:C3. 期权的卖方承担的义务是()。
A. 强制买方履行合约B. 强制卖方履行合约C. 选择是否履行合约D. 无条件履行合约答案:D4. 期权的内在价值是指()。
A. 期权合约的购买价格B. 期权合约的执行价格C. 期权合约的市场价格D. 期权合约的执行价格与标的资产市场价格之差答案:D5. 期权的时间价值是指()。
A. 期权合约的购买价格B. 期权合约的执行价格C. 期权合约的市场价格与内在价值之差D. 期权合约的市场价格答案:C二、多选题6. 期权的类型包括()。
A. 看涨期权B. 看跌期权C. 欧式期权D. 美式期权答案:A, B7. 影响期权价格的因素包括()。
A. 标的资产价格B. 期权的执行价格C. 期权的到期时间D. 市场利率答案:A, C, D8. 期权策略中,以下哪些属于对冲策略()。
A. 保护性看跌期权B. 买入看涨期权C. 卖出看跌期权D. 卖出看涨期权答案:A, D三、判断题9. 期权的到期日是期权合约中规定的最后交易日。
()答案:正确10. 期权的杠杆效应是指期权合约的价格变动幅度通常大于标的资产的价格变动幅度。
()答案:正确四、简答题11. 什么是期权的行权?答案:期权的行权是指期权的买方在期权合约到期前或到期日,按照期权合约规定的执行价格,购买(对于看涨期权)或出售(对于看跌期权)标的资产的权利。
五、案例分析题12. 假设投资者购买了一份执行价格为50美元的看涨期权,期权费为2美元,期权到期时,标的资产的市场价格为55美元。
请问该投资者是否应该行权?如果行权,其盈利情况如何?答案:投资者应该行权。
因为标的资产的市场价格(55美元)高于执行价格(50美元)加上期权费(2美元),即52美元。
期货期权综合题

综合题1.3月10日,某交易所5月份小麦期货合约的价格为7.65美元/蒲式耳,7月份小麦合约的价格为7.50美元/蒲式耳。
某交易者如果此时人市,采用熊市套利策略(不考虑佣金成本),那么下面选项中能使其亏损最大的是5月份小麦合约的价格()。
A.涨至7.70美元/蒲式耳,7月份小麦合约的价格跌至7.45美元/蒲式耳B.跌至7.60美元/蒲式耳,7月份小麦合约的价格跌至7.40美元/蒲式耳C.涨至7.70美元/蒲式耳,7月份小麦合约的价格涨至7.65美元/蒲式耳D.跌至7.60美元/蒲式耳,7月份小麦合约的价格涨至7.55美元/蒲式耳2. 投资者以65000元/吨卖出一手8月铜期货合约,同时以63000元/吨买入一手10月铜合约,当8月和10月铜合约价差为()元/吨时,该投资者亏损。
A. 2100B. 1500C. 1000D. -3003. 某客户开仓卖出大豆期货合约20手,成交价格为2020元/吨,当天平仓5手合约,交价格为2030元,当日结算价格为2040元/吨,则其当天平仓盈亏为成____元,持仓盈亏为____元。
()A.-500;-3000B.500;3000C.-3000;-50D.3000;5004. 5月15日,某交易所8月份黄金期货合约的价格为399.5美元/盎司,10月份黄金期货合约的价格为401美元/盎司。
某交易者此时入市,买人一份8月份黄金期货合约,同时卖出一份10月份黄金期货合约。
在不考虑其他因素影响的情况下,则下列选项中能使该交易者盈利最大的是( )。
A.8月份黄金合约的价格涨至402.5美元/盎司,10月份黄金合约的价格涨至401.5美元/盎司B.8月份黄金合约的价格降至398.5美元/盎司,10月份黄金合约的价格降至399.5美元/盎司C.8月份黄金合约的价格涨至402.5美元/盎司,l0月份黄金合约的价格涨至404.00美元/盎司D.8月份黄金合约的价格降至398.5美元/盎司,10月份黄金合约的价格降至397.00美元/盎司5.5月20日,某交易者买人两手10月份铜期货合约,价格为16950元/吨,同时卖出两手12月份铜期货合约,价格为17020元/吨,两个月后的7月20日,10月份铜合约价格变为17010元/吨,而12月份铜合约价格变为17030元/吨,则5月20日和7月20日相比,两合约价差( )元/吨。
宁夏省期货从业资格 期权考试试题

宁夏省期货从业资格:期权考试试题一、单项选择题1、期权交易中,买方可以()期权。
A.买入看涨B.卖出看涨C.买入看跌D.卖出看跌2、在期权交易中,当市场价格与期权行权价格一致时,期权()。
A.不会对买卖双方产生影响B.对买卖双方均产生影响C.只对买方产生影响D.只对卖方产生影响3、期权交易的标的物是()。
A.期货合约B.期权合约C.股票或债券D.以上都不是二、多项选择题1、期权的主要功能包括()。
A.规避风险B.保险作用C.套期保值D.价格发现2、期权交易的意义在于()。
A.为投资者提供高收益的可能性B.可以进行杠杆交易,提高资金使用效率C.可以对冲风险,降低投资的不确定性D.可以进行套期保值,锁定未来价格3、下列哪些是期权的买方?()A.持有期权合约的多头方B.卖出期权合约的空头方C.行权后持有期货合约的多头方D.行权后持有期货合约的空头方4、下列哪些是期权的卖方?()A.持有期权合约的空头方B.卖出期权合约的多头方C.行权后持有期货合约的多头方D.行权后持有期货合约的空头方5、在期权交易中,期权的权利金是指()。
A.期权的价格B.期权的行权价格C.期权的内在价值D.期权的持仓价差6。
在期权交易中,当市场价格与期权行权价格一致时,期权买方可以()。
A。
以行权价格买入标的物 B。
以行权价格卖出标的物 C。
行使权利 D。
不可以行使权利7。
在期权交易中,当市场价格与期权行权价格一致时,期权卖方可以()。
A。
以行权价格买入标的物 B。
以行权价格卖出标的物 C。
行使权利 D。
不可以行使权利8。
期权交易的标的物是()。
A。
期货合约 B。
期权合约C。
股票或债券 D。
以上都不是9。
下列哪些是期权的买方?()A。
持有期权合约的多头方 B。
卖出期权合约的空头方 C。
行权后持有期货合约的多头方 D。
行权后持有期货合约的空头方10。
下列哪些是期权的卖方?()A。
持有期权合约的空头方 B。
卖出期权合约的多头方 C。
行权后持有期货合约的多头方 D。
2024年度精选期货与期权题库及答案

负责提供交易场所、设施及相关服务,制定并实施业务规则,对市 场参与者进行自律管理。
期货业协会
负责行业自律管理,制定行业标准和业务规范,推动行业诚信建设 和创新发展。
25
从业人员资格要求和行为规范
从业人员资格要求
通过期货从业人员资格考试,取得相 应资格证书。
从业人员行为规范
遵守法律法规和职业道德规范,勤勉 尽责,保护投资者合法权益。
2024/3/24
21
保持良好心态,避免过度交易
保持冷静
在交易中保持冷静和理性,不被市场情绪左 右,避免冲动交易。
学会等待
耐心等待良好的交易机会出现,不盲目追求 交易次数和频率。
2024/3/24
控制情绪
把交易当作一项事业来经营,保持平和的心 态,不被短期波动所影响。
22
05
法律法规与监管政策解读
2024/3/24
9
技术分析方法
01
02
03
趋势线分析
通过绘制趋势线,判断市 场趋势的走向,以及趋势 的强弱和可能发生的转折 。
2024/3/24
形态分析
识别各种价格形态,如头 肩顶、双底等,预测未来 价格的变动方向和幅度。
量价关系分析
结合成交量和持仓量的变 化,判断市场主力的意图 和未来价格的动向。
32
感谢您的观看
THANKS
2024/3/24
33
2024/3/24
23
国内外相关法律法规概述
国内期货与期权相关法律法规
《期货交易管理条例》、《期货公司监督管理办法》等。
国际期货A)主协议等。
2024/3/24
24
监管机构及其职责
期权与期货期末考试试题

期权与期货期末考试试题# 期权与期货期末考试试题## 一、选择题(每题2分,共20分)1. 期权的内在价值是指:A. 期权的市场价格B. 期权的执行价格C. 期权的执行价格与标的资产市场价格之差D. 期权的执行价格与标的资产市场价格之和2. 期货合约的交割方式通常包括:A. 现金结算B. 实物交割C. 延期交割D. 以上都是3. 以下哪项不是期权的时间价值:A. 期权的到期时间B. 标的资产的波动性C. 期权的执行价格D. 无风险利率4. 期货合约的保证金分为:A. 初始保证金和维持保证金B. 初始保证金和交易保证金C. 维持保证金和交易保证金D. 初始保证金和结算保证金5. 期权的杠杆效应是指:A. 期权价格变动与标的资产价格变动的比率B. 期权价格与标的资产价格的比率C. 期权的内在价值与标的资产价格的比率D. 期权的执行价格与标的资产价格的比率## 二、简答题(每题10分,共20分)1. 简述期权与期货的主要区别。
2. 解释什么是看涨期权和看跌期权,并给出它们的基本特征。
## 三、计算题(每题15分,共30分)1. 假设你持有一份执行价格为50美元的看涨期权,标的资产的当前市场价格为60美元,无风险利率为5%,期权的到期时间为6个月。
请计算该期权的内在价值和时间价值。
2. 假设你购买了一份期货合约,合约规模为100单位,当前市场价格为每单位100美元,你需要支付的初始保证金为2000美元。
如果市场价格下跌到每单位90美元,你需要追加多少保证金?## 四、案例分析题(每题15分,共30分)1. 某投资者购买了一份看跌期权,执行价格为100美元,期权费为5美元。
当标的资产的市场价格下跌到90美元时,投资者选择行使期权。
请分析该投资者的盈亏情况。
2. 某公司预计未来需要购买大量原材料,为避免价格上涨,决定使用期货合约进行套期保值。
请分析该公司使用期货合约进行套期保值的优缺点。
## 五、论述题(15分)论述期权定价模型Black-Scholes模型的基本假设及其在实际应用中的局限性。
2023年上海期货从业资格期权交易的基本策略考试试题

上海期货从业资格:期权交易旳基本方略考试试题一、单项选择题(共25题,每题2分。
每题旳备选项中,只有一种最符合题意)1、自然人投资者甲向期货企业会员乙申请开立股指期货交易编码,乙对甲进行了审查,发现甲有若干地方不太符合原则,于是期货企业会员乙合适调整了一下对投资者旳合适性原则,给甲开立了股指期货交易编码。
[根据上述资料,请回答如下问题。
]甲申请股指期货交易编码应当具有旳条件是__。
A.净资产不低于人民币100万元B.申请开户时保证金账户可用资金余额不低于人民币50万元C.不存在不良诚信记录D.具有合计10个交易日、20笔以上旳股指期货仿真交易成交记录,或者近来3年内具有15笔以上旳商品期货交易成交记录2、法规、政策规定向投资者承诺或保证收益,情节严重旳,由中国期货业协会()A.暂停从业人员资格6个月至12个月B.撤销期货从业人员资格并在2年内拒绝受理其从业人员资格申请C.撤销期货从业人员资格并在3年内或永久性拒绝受理其从业人员资格申请D.公开通报批评3、期货投资者保障基金产生旳利息以及运用所产生旳多种收益等孳息归属()。
A.期货交易所B.中国证监会C.期货投资者保障基金D.风险准备金4、林某是甲期货企业旳期货从业人员,在从业过程中,林某为了获得更多客户,在为客户提供服务过程中,多次向客户谎称其竞争对手——乙期货企业信誉低下,常常欺骗客户等,致使乙期货企业业务大幅度下滑。
对于林某旳行为,期货业协会有权根据详细状况予以()旳惩戒。
A.训诫B.公开训斥C.撤销其从业资格D.行政惩罚5、成交量和持仓量等,对未来期货价格旳走势进行旳判断分析措施。
A.心理分析B.技术分析C.基本分析D.价值分析6、孙某在期货企业里面持续担任董事长、副总经理分别达5年、4年之久,张某已经获得了期货从业人员资格。
由于期货行情稳定,形势良好,该期货企业旳资本迅速扩大,到达1.5亿元,于是该期货企业开始申请金融期货全面结算会员资格。
期权考试题库及答案2024

期权考试题库及答案2024一、单项选择题(每题2分,共20分)1. 期权合约的买方拥有的权利是:A. 购买权B. 出售权C. 购买或出售权D. 无权答案:C2. 欧式期权只能在到期日执行,以下哪项描述正确?A. 正确B. 错误C. 无法确定D. 以上都不对答案:A3. 期权的时间价值随着到期日的临近而:A. 增加B. 减少C. 不变D. 先增加后减少答案:B4. 以下哪项不是期权的基本类型?A. 看涨期权B. 看跌期权C. 双向期权D. 以上都是答案:C5. 期权的内在价值是指:A. 期权的市场价格B. 期权的执行价格C. 期权的执行价格与标的资产市场价格之间的差额D. 期权的时间价值答案:C6. 期权的杠杆效应是指:A. 期权价格变动与标的资产价格变动的比率B. 期权的内在价值C. 期权的时间价值D. 期权的执行价格答案:A7. 期权的到期日是指:A. 期权合约的开始日期B. 期权合约的结束日期C. 期权合约的交割日期D. 期权合约的结算日期答案:B8. 期权的执行价格是指:A. 期权合约的购买价格B. 期权合约的出售价格C. 期权合约规定的标的资产交易价格D. 期权合约的市场价格答案:C9. 期权的卖方承担的义务是:A. 购买标的资产B. 出售标的资产C. 根据买方的选择购买或出售标的资产D. 无义务答案:C10. 期权的行权是指:A. 期权的购买B. 期权的出售C. 期权的执行D. 期权的放弃答案:C二、多项选择题(每题3分,共15分)1. 以下哪些因素会影响期权的价格?A. 标的资产价格B. 执行价格C. 到期时间D. 无风险利率答案:A, C, D2. 期权的时间价值受以下哪些因素影响?A. 标的资产的波动性B. 期权的执行价格C. 到期时间D. 无风险利率答案:A, C3. 以下哪些是期权的内在价值可能为零的情况?A. 看涨期权的执行价格等于标的资产市场价格B. 看跌期权的执行价格高于标的资产市场价格C. 看涨期权的执行价格低于标的资产市场价格D. 看跌期权的执行价格等于标的资产市场价格答案:A, B4. 以下哪些是期权的卖方需要考虑的风险?A. 标的资产价格的波动B. 期权的时间价值C. 期权的内在价值D. 期权的执行价格答案:A, C5. 以下哪些是期权的买方可能面临的风险?A. 期权的时间价值损失B. 期权的内在价值损失C. 期权的执行价格变动D. 标的资产价格的波动答案:A, D三、判断题(每题1分,共10分)1. 期权的买方在任何情况下都不会亏损超过支付的期权费。
期货从业:期权考试题

期货从业:期权考试题1、判断题如果看跌期权的买方将该期权平仓,则该买方将变为看跌期权的卖方。
()正确答案:错参考解析:看跌期权的卖方是指开仓时卖出看跌期权的交易者,如果看跌期权的买方将该期权平仓,只是执行了看跌(江南博哥)期权的买方的权利,不会转变为期权的卖方。
2、单选权利金的最终确定是经过期权买卖双方的经纪人在交易大厅通过()方式形成。
A.私下协商价格B.由交易所确定标准化价格C.公开竞价D.集合竞价正确答案:C参考解析:期货与期货期权交易都是在期货交易所内通过公开竞价的方式进行,交易达成后都必须通过结算所统一结算。
3、判断题期权交易的绝大部分均是通过履约平仓的方式进行的。
()正确答案:错4、判断题期权卖方取得的是买卖的权利,而不负有必须买进或卖出的义务;卖方有执行的权利,也有不执行的权利,完全可以灵活选择。
()正确答案:错参考解析:期权买方取得的是买卖的权利,而不负有必须买进或卖出的义务;买方有执行的权利,也有不执行的权利,完全可以灵活选择。
5、单选在看跌期权中,如果买方要求执行期权,则买方将获得标的期货合约的()部位。
A.多头B.空头C.开仓D.平仓正确答案:B参考解析:在期货期权交易中,只有期权买方有权在期权合约规定时间要求行权,即可以执行价格获得一个期货头寸。
看涨期权的买方成为期货交易的多头,卖方成为空头;看跌期权的买方成为期权交易的空头,卖方成为多头。
6、判断题与期货交易相比,期权买方可以为投资者提供更大的杠杆效应。
()正确答案:对7、单选按照买方权利的不同,期权可分为()。
A.看涨期权和看跌期权B.现货期权和远期期权C.现货期权和期货期权D.远期期权和期货期权正确答案:A参考解析:根据买方权利的不同,期权可分为看涨期权、看跌期权。
看涨期权买方将来有权按约定价格买进标的资产,看跌期权买方将来有权按约定价格卖出标的资产;按照期权合约标的物的不同,期权可以分为现货期权和期货期权。
8、判断题看跌期权买方的交易对手就是看涨期权的卖方。
期货期权选择试题及答案

期货期权选择试题及答案一、单选题(每题2分,共20分)1. 期货合约是一种标准化的:A. 远期合约B. 期权合约C. 互换合约D. 买卖协议答案:A2. 在期权交易中,买方拥有的权利是:A. 出售标的资产B. 购买标的资产C. 强制卖出标的资产D. 强制购买标的资产答案:B3. 期货合约的主要功能不包括以下哪一项?A. 风险管理B. 价格发现C. 投机D. 增加市场流动性答案:D4. 以下哪个不是期权的基本类型?A. 看涨期权B. 看跌期权C. 双向期权D. 欧式期权答案:C5. 期货交易中的保证金通常分为哪两种?A. 初始保证金和维持保证金B. 初始保证金和变动保证金C. 维持保证金和变动保证金D. 固定保证金和变动保证金答案:A6. 期权的时间价值是指:A. 期权合约的内在价值B. 期权合约的市场价格C. 期权合约的外在价值D. 期权合约的理论价值答案:C7. 期货合约的交割方式通常包括:A. 现金结算和实物交割B. 只有现金结算C. 只有实物交割D. 期货合约不允许交割答案:A8. 下列哪个因素不影响期权的内在价值?A. 标的资产的当前价格B. 期权的执行价格C. 期权的到期时间D. 市场利率答案:D9. 期货合约的杠杆效应是指:A. 投资者可以控制比实际投资金额更大的资产B. 期货合约的价格变动幅度比标的资产大C. 期货合约的价格变动幅度比标的资产小D. 期货合约的价格总是高于标的资产的价格答案:A10. 在期权交易中,卖方的主要风险是:A. 无限亏损B. 有限亏损C. 零亏损D. 无法预测的亏损答案:A二、多选题(每题3分,共15分)11. 以下哪些因素可能影响期权的时间价值?A. 期权的到期时间B. 标的资产的波动性C. 市场利率D. 期权的执行价格答案:A, B, C12. 期货交易中,以下哪些行为可能导致保证金的增加?A. 市场价格的不利变动B. 合约的杠杆比率增加C. 交易所规定的保证金水平提高D. 投资者的信用评级下降答案:A, C13. 以下哪些属于期权交易的基本策略?A. 买入看涨期权B. 卖出看跌期权C. 跨式期权D. 期货合约答案:A, B, C14. 在期货市场中,以下哪些行为可以视为投机?A. 通过期货合约对冲现货价格波动的风险B. 预测市场价格变动并进行买卖以期获利C. 通过期货合约进行套利交易D. 通过期货合约进行套期保值答案:B, C15. 以下哪些因素会影响期货合约的理论价格?A. 标的资产的当前价格B. 期货合约的到期时间C. 无风险利率D. 标的资产的预期股息答案:A, B, C, D三、判断题(每题1分,共10分)16. 期权的内在价值是指期权的市场价格。
期权练习试卷3(题后含答案及解析)

期权练习试卷3(题后含答案及解析)题型有:1.1.期货价格可能高于,也可能低于现货价格,二者之间的差异主要是取决于( )Ⅰ利率水平Ⅱ资产收益率Ⅲ储藏费Ⅳ保险费A.全部B.ⅠⅡⅢC.ⅡⅢⅣD.ⅠⅢⅣ正确答案:A 涉及知识点:期权2.有关期货价格与远期价格之间的关系,以下说法正确的是( )A.期货价格与远期价格之间的差额为基差B.现实情况下,当利率水平不可预测地变动时,远期价格与期货价格在理论上应不会相等C.在任何条件下,某交割日期的合约的远期价格与具有相同交割日期的类似合约的期货价格完全相同D.当基础资产的价格S与利率呈强负相关关系时,期货价格将高于远期价格正确答案:B 涉及知识点:期权3.当基础资产的现货价格S与利率存在强正相关关系时,期货价格( )远期价格A.高于B.低于C.等于D.不能确定正确答案:A 涉及知识点:期权4.假设一只股票当前市价为40元,预期在未来2年内不会支付股息;2年期无风险利率为5%(连续复利)。
以5%的年利率借入4000元购入该只股票100股,期限为2年,订立远期合约,约定2年后以每股45元的交割价格卖出其100股股票,套利者可以获得无风险利润( )元A.54B.63C.79D.89正确答案:C 涉及知识点:期权5.假设一份6个月的远期合约,其基础资产以每年4%的收益率支付收益。
无风险年利率为10%(连续复利计算)。
资产现价25元,合约远期价格为( )元A.21.74B.23.64C.25.76D.27.76正确答案:C 涉及知识点:期权6.假设一张一年期黄金期货合约。
储藏黄金的费用为2元/盎司(该费用在年终支付);黄金现货价格为450元,所有期限的无风险利率均为每年7%(连续复利)。
如果合约远期价格为800元,则对于一年期期货,投资者应该( ) A.保持空头B.保持多头C.保持零头寸D.不能确定正确答案:A 涉及知识点:期权7.期权也称选择权,是指某一标的物的买权或者卖权,具有在某一限定时间内按某一指定的价格买进或卖出某一特定商品或合约的权利。
期货基础知识:期权试题及答案(题库版)

期货基础知识:期权试题及答案(题库版)1、判断题期权头寸的建立包括买入开仓和卖出开仓。
()正确答案:对参考解析:期权头寸的建立即开仓,包括买入开仓和卖出开仓。
买入开仓者称为期权合约的多头,卖出开仓者被称为期权合约的空头,(江南博哥)所以题目表述正确。
2、单选期权多头方支付一定费用给期权空头方,作为拥有这份权利的报酬。
这笔费用称为()。
A.权利金B.保证金C.交易佣金D.协定价格正确答案:A3、单选若某投资者11月份以400点的权利金卖出1份执行价格为15000点的12月份恒指看涨期权;同时,又以200点的权利金卖出1份执行价格为15000点的12月份恒指看跌期权,则该投资者的最大收益是()点。
A.400B.200C.600D.100正确答案:C参考解析:期权卖出方的最大收益是全部权利金,即当两份期权都不执行,即恒指等于15000点时,该投资者的收益达到最大=400+200=600(点)。
4、判断题期权的买方预期标的物市场价格下跌而买入看跌期权,标的物市场价格下跌越多,买方行权可能性越大,行权卖出标的物后获取收益的可能性越大、获利可能越多。
()正确答案:对5、单选关于期货看涨期权的说法中,正确的是()。
A.时间价值=内涵价值B.时间价值=保证金C.时间价值=权利金+内涵价值D.时间价值=权利金-内涵价值正确答案:D6、单选期货交易与期权交易相比,相同之处是()。
A.买卖双方的权利和义务相同B.买卖双方都需要缴纳保证金C.买卖双方的风险和收益一致D.交易的对象都是标准化合约正确答案:D7、判断题看跌期权卖方的盈亏曲线与看跌期权买方的盈亏曲线是对称的。
()正确答案:对8、判断题一般来说,执行价格与市场价格的差额越大,则时间价值就越小。
当一种期权处于极度实值或极度虚值时,其时间价值都将为零。
()正确答案:对9、单选只能在期权到期日行使权利的期权是()。
A.美式期权B.欧式期权C.看跌期权D.看涨期权正确答案:B10、判断题目前,全球的期权交易从最初的股票扩展到包括大宗农副产品、债券、股指等金融产品,外汇以及黄金白银在内的近100个品种。
期货基础知识:期权测试题(题库版)

期货基础知识:期权测试题(题库版)1、单选1973年4月26日,期权市场发生了历史性的变化,一个以股票为标的物的期权交易所,()成立,这堪称是期权发展史中划时代意义的事件,标志着现代意义的期权市场的诞生。
A.CBO(江南博哥)TB.CMEC.LIFFED.CBOE正确答案:D2、多选下列说法中,错误的有()。
A.期货公司应当每半年向公司全体董事提交书面报告,说明净资本等各项风险监管指标的具体情况,该书面报告应当经期货公司财务负责人签字确认B.期货公司应当每半年向公司全体董事提交书面报告,说明净资本等各项风险监管指标的具体情况,该书面报告应当经期货公司法定代表人签字确认C.期货公司应当每半年向公司全体股东提交书面报告,说明净资本等各项风险监管指标的具体情况,该书面报告应当经全体董事签字确认,并应当取得股东的签收确认证明D.期货公司应当每半年向公司全体股东提交书面报告,说明净资本等各项风险监管指标的具体情况,该书面报告应当经期货公司法定代表人签字确认,并应当取得股东的签收确认证明正确答案:A, D3、多选与场内期权相比,场外期权具有的特点是()。
A.合约非标准化B.交易品种多样、形式灵活、规模巨大C.交易对手机构化D.流动性风险和信用风险小正确答案:A, B, C4、判断题买入期权建仓投机和卖出期权建仓投机所面临的风险和收益具有不对称性,前者面临的风险有限,最大可能的损失是权利金,后者最大可能的收益是权利金,而可能面临的风险较大。
()正确答案:对5、判断题1982年芝加哥期货交易所推出了美国长期国债期货期权合约,标志着金融期货期权的诞生,引发了期货交易的又一场革命。
()正确答案:对6、单选在期权交易中,()是场内期权合约中唯一能在交易所内讨价还价的要素,其他合约要素均已标准化。
A.执行价格B.合约到期日C.履约日D.期权权利金正确答案:D参考解析:场内期权合约是由交易所统一制定的标准化合约,执行价格、合约到期日、履约日等均为规定好的,只有期权权利金由双方共同决定。
期权期货考试大题
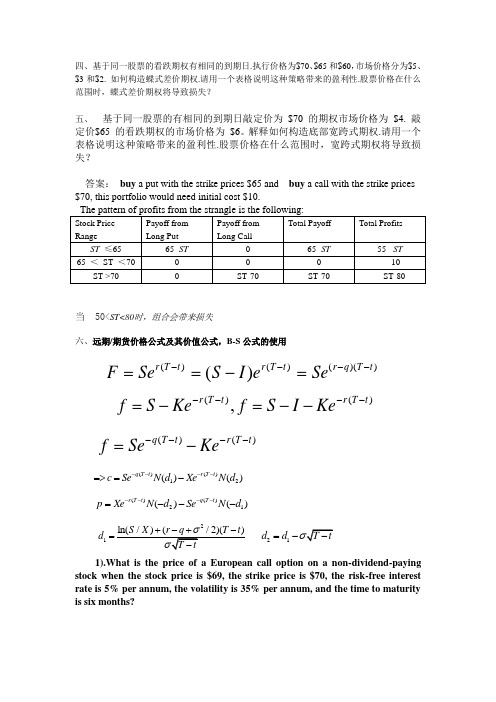
四、基于同一股票的看跌期权有相同的到期日.执行价格为$70、$65和$60,市场价格分为$5、$3和$2. 如何构造蝶式差价期权.请用一个表格说明这种策略带来的盈利性.股票价格在什么范围时,蝶式差价期权将导致损失?五、 基于同一股票的有相同的到期日敲定价为 $70的期权市场价格为 $4. 敲定价$65 的看跌期权的市场价格为 $6。
解释如何构造底部宽跨式期权.请用一个表格说明这种策略带来的盈利性.股票价格在什么范围时,宽跨式期权将导致损失?答案: buy a put with the strike prices $65 and buy a call with the strike prices $70, this portfolio would need initial cost $10.当 50<ST<80时,组合会带来损失六、远期/期货价格公式及其价值公式,B-S 公式的使用()()12()()q T t r T t c Se N d Xe N d ----=>=- ()()21()()r T t q T t p Xe N d Se N d ----=---21d = 21d d =-1).What is the price of a European call option on a non-dividend-paying stock when the stock price is $69, the strike price is $70, the risk-free interest rate is 5% per annum, the volatility is 35% per annum, and the time to maturity is six months?()()()()()r T t r T t r q T t F Se S I e Se----==-=)()(t T r t T q KeSe f -----=)()(,t T r t T r KeI S f Ke S f ------=-=2). Suppose the current value of the index is 500, continuous dividend yields of index is 4% per annum, the risk-free interest rate is 6% per annum . if the price of three-month European index call option with exercise price 490is $20, What is the price of a three-month European index put option with exercise price 490?by put-call parity3) What is the price of a European futures put option :current futures price is $19, the strike price is $20, the risk-free interest rate is 12% per annum, the volatility is 20% per annum, and the time to maturity is five months? (保留2位小数)Solution: In this case F=19,X=20, r=0.12, σ=0.20, T -t=0.42,210.33d ==-210.46d d =-=-(0.33)0.6293,(0.46)0.6772N N ==12()(0.33)0.6293,()(0.46)0.6772N d N N d N -==-==The price of the European put is()()210.120.420.120.42()()200.6772190.6293 1.51r T t r T t p Xe N d Fe N d ee-----⨯-⨯=---=⨯-⨯=4) A one-year-long forward contract on a non-dividend-paying stock is entered into when the stock price is $40 and the risk-free rate of interest is 10% per annum with continuous compounding.(a) What are the forward price and the initial value of the forward contract?(b) Six months later, the price of the stock is $45 and the risk-free interest rate isstill 10%. What are the forward price and the value of the forward contract? The forward price, 21.44401.0)(===-e Se F t T r ,The initial value of the forward contract is zero.0=f (a) The delivery price K in the contract is $44.21. The value of the forward contract after six months is given: 95.221.44455.01.0)(=-=-=⨯---e Ke S f t T r The forward price, 31..47455.01.0)(===⨯-e Se F t T r七 Consider a portfolio that is delta neutral, with a gamma of -5,000 and a vega of -8,000. Suppose that a traded option has a gamma of 0.5, a vega of 2.0, and a delta of 0.6.Another traded option with a gamma of 0.8, a vega of 1.2, and a delta of 0.5.What position in the traded two call options and in the underlying asset would make the portfolio gamma ,vega and delta neutral ?Solution: If , w1 ,w2 , ,w3 are the amounts of the two traded options and underlying asset included in the portfolio, we require that -5,000 + 0.5w1 + 0.8 w2 = 0 - 8,000 + 2.0w1 + 1.2w2 = 0 w3 +0.6w1 + 0.5 w2 =0=> w1 = 400, w2 = 6,000, w3 =-3240.=>The portfolio can be made gamma,vega and delta neutral by including long: (1) 400 of the first traded option(2) 6,000 of the second traded option.And short 3240 underlying asset.八 1)证明在风险中性环境下,到期的欧式看涨期权被执行的概率为 )(2d N ,2) 使用风险中性定价原理,假设股票1的价格和股票2的价格分别服从几何布朗运动,且独立,给到期损益为如下形式的欧式衍生品定价:121,2: 0 elseT T T K S X S X T f ⎧>>⎪=⎨⎪⎩ Solution: Since ())(),)2/(ln ~ln 22t T t T r S N S T ---+σσ()N(d )))(2/(ln ln ( )))(2/(ln ln (1)ln p(ln 1)ln p(ln )p(222=------=------=<-=>=>tT t T r S X N tT t T r S X N X S X S X S T T T σσσσSince 121,22 : and p() N(d )0 elseT T T T K S X S X T f S X ⎧>>⎪=>=⎨⎪⎩121,2212122122()()2122[] P()K[P() *P() ]K[N(d )*N(d )][][N(d )*N(d )]T T T T T r T t r T t T E f K S X S X S X S X f e E f e K ----=>>=>>=== Where1221d =2222d =,九、Use two-step tree to value an American 2-year put option on anon-dividend-paying stock, current stock price is 50, the strike price is $52, and the volatility of stock price is 30% per annum, the risk-free interest rate is 5% per annum. (保留2位小数)In this case, S=50, X = 52,σ = 0.3,Δt =1, r=0.05 , the parameters necessary to construct the tree are11.35,0.74u e du====, 0.05*1=1.10e0.05*10.51,10.49e dp pu d-==-=-{}])1([,max.11.1,jijitrjijjifppfedSuXf+++∆---+-=十If a stock price, S, follows geometric Brownian motiontSdWSdtdSσμ+=1) What is the process followed by the variable n S? Show that n S also follows geometric Brownian motion.2)The expected value of ST is =)(TSE)(tTSe-μ. What is the expected value ofnTS?3) The varaince of ST is =)(TSD)1()()(222---tTtT eeSσμ.What is the variance of nTS?4) Using risk-neutral valuation to value the derivative, whose payoff at maturity is507.43 50227.4424.5691.1137.0414.9667.40.93:n T TT f S = 1)We now use Ito's lemma to derive the process followed by n S , Define n S G =,0,)1(,2221=∂∂-=∂∂=∂∂--tGS n n SG nS SGn n t SdW Sdt dS σμ+=222)(21dS S G dS S G dt t G dG ∂∂+∂∂+∂∂= 221)()1(21)(t n t n SdW Sdt S n n SdW Sdt nS σμσμ+-++=--dt S n n dW S n dt S n n t n n 2)1(21σσμ-++=t n n n dW S n dt S n n S n σσμ+-+=])1(21[2t n n dW S n dt S n n n dG σσμ+-+=])1(21[2t GdW n Gdt n n n σσμ+-+=])1(21[2n S G =So that n S also follows geometric Brownian motion. 2)t SdW Sdt dS ceσμ+=sin=)(T S E )(t T Se -μ.dG t GdW n Gdt n n n σσμ+-+=])1(21[2 =⇒)(T G E )]()1(21[2t T n n n Ge--+σμ n S G = ,=∴)(n T S E )]()1(21[2t T n n n neS --+σμ3) Since t SdW Sdt dS σμ+= and varaince of ST is=)(T S D )1()()(222---t T t T e e S σμ.Similarly, by dG t GdW n Gdt n n n σσμ+-+=])1(21[2We get the varaince of nT S is=)(nT S D =)(T G D ]1[)()]()1(2[2222----+t T n t T n n n e e G σσμ]1[)()()]()1(2[2222-=---+t T n t T n n n n ee S σσμ十一、 In a risk-neutral world, suppose stock prices follow geometric Brownian motion,dS rSdt SdW σ=+1) What is the process followed by the variable n S by Ito’s lemma? Show thatn S also follows geometric Brownian motion.2) The expected value of T S is =)(T S E ()r T t Se -. What is the expected value ofnT S ?4) Using risk-neutral valuation to value the derivative, whose payoff at maturity is:n T T at T f S =十二、Consider the price of a stock, S , which is the following processt dW dt dS σμ+=where t W is a standard Brownian motion. For the first three years,5,211==σμ; for the next three years, 4,322==σμ. If the initial value ofstock price is $10, what is the expect value of the stock price at the end of year 6? The change in S during the first three years has the probability distribution 21~(23,53)(6,75)S N N ∆⨯⨯=The change in S during the next three years has the probability distribution )48,9()34,33(~22N N S =⨯⨯∆ The probability distribution of the change is therefore12~(15,123)S S S N ∆=∆+∆012T S S S S =+∆+∆Since the initial value of the variable is 10,01225T ES ES E S E S =+∆+∆=, 127548123T DS D S D S =∆+∆=+=~(25,123)T S Nthe expect value of the stock price at the end of year 6 is 25.。
期权知识考试题库

期权知识考试题库一、单选题1、期权合约中规定的未来买入或卖出标的资产的价格称为()A 执行价格B 市场价格C 权利金D 结算价格答案:A解析:执行价格是期权合约中规定的未来买入或卖出标的资产的价格。
2、以下关于期权权利金的说法,错误的是()A 权利金是期权买方为获得期权合约所赋予的权利而向卖方支付的费用B 权利金是买方可能的最大损失C 权利金是卖方可能的最大收益D 权利金由内涵价值和时间价值组成答案:B解析:权利金是买方为获得期权合约所赋予的权利而向卖方支付的费用,是卖方可能的最大收益。
买方的最大损失为支付的权利金,但不是唯一可能的损失。
权利金由内涵价值和时间价值组成。
3、对于看涨期权,当标的资产的市场价格()执行价格时,期权买方会行权。
A 高于B 低于C 等于D 以上都有可能答案:A解析:看涨期权买方行权的条件是标的资产的市场价格高于执行价格。
4、看跌期权的买方拥有在约定期限内按()卖出一定数量标的资产的权利。
A 执行价格B 市场价格C 权利金D 结算价格答案:A解析:看跌期权的买方拥有在约定期限内按执行价格卖出一定数量标的资产的权利。
5、期权的时间价值与()因素无关。
A 标的资产价格波动率B 期权到期时间C 标的资产价格D 行权价格答案:D解析:期权的时间价值与标的资产价格波动率、期权到期时间、标的资产价格等因素有关,与行权价格无关。
二、多选题1、以下属于期权基本要素的有()A 标的资产B 执行价格C 到期日D 权利金答案:ABCD解析:期权的基本要素包括标的资产、执行价格、到期日和权利金。
2、影响期权价格的因素包括()A 标的资产价格B 行权价格C 标的资产价格波动率D 无风险利率E 到期时间答案:ABCDE解析:标的资产价格、行权价格、标的资产价格波动率、无风险利率和到期时间都会影响期权价格。
3、期权按照行权方向可以分为()A 看涨期权B 看跌期权C 欧式期权D 美式期权答案:AB解析:期权按照行权方向分为看涨期权和看跌期权;欧式期权和美式期权是按照行权时间来分类的。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
d1
ln(F
/
X)
( 2 / T t
2)(T
t)
0.33
d2 d1 0.2 0.4167 0.46
N(0.33) 0.6293, N(0.46) 0.6772
N (d1) N (0.33) 0.6293, N (d2 ) N (0.46) 0.6772
In this case, S=50, X = 52,σ = 0.3, Δt =1, r=0.05 , the parameters necessary to construct the tree are
u e t 1.35, d 1 0.74 , e0.05*1=1.10 p e0.05*1 d 0.51, 1 p 0.49
(a) What are the forward price and the initial value of the forward contract? (b) Six months later, the price of the stock is $45 and the risk-free interest rate is
still 10%. What are the forward price and the value of the forward contract?
The forward price, F Ser(Tt) 40e0.1 44.21,
The initial value of the forward contract is zero. f 0
f er(T t) E[ fT ] er(T t) K[N(d21 ) * N(d22 )]
d21
ln(S1
/
X1) (r 12 1 T t
/
2)(T
t)
, d22
ln(S 2
/
X
2
)
(r
2 2
2 T t
/
2)(T
t)
,
九、Use two-step tree to value an American 2-year put option on a
(1) 400 of the first traded option (2) 6,000 of the second traded option. And short 3240 underlying asset.
八 1)证明在风险中性环境下,到期的欧式看涨期权被执行的概率为 N (d2 ) ,
2) 使用风险中性定价原理,假设股票 1 的价格和股票 2 的价格分别服从几何布朗运动,且 独立,给到期损益为如下形式的欧式衍生品定价:
答案: buy a put with the strike prices $65 and buy a call with the strike prices
$70, this portfolio would need initial cost $10.
The pattern of profits from the strangle is the following:
The price of the European put is p Xer(T t) N (d2 ) Fer(T t) N (d1) 20e0.120.42 0.6772 19 0.6293e0.120.42 1.51
4) A one-year-long forward contract on a non-dividend-paying stock is entered into when the stock price is $40 and the risk-free rate of interest is 10% per annum with continuous compounding.
Stock Price
Payoff from
Payoff from
Total Payoff
Total Profits
Range
Long Put
Long Call
ST ≤65
65- ST
0
65- ST
55 - ST
65 < ST <70
0
0
0
-10
ST >70
0
ST-70
ST-70
ST-80
当 50<ST<80时,组合会带来损失
N (
ln
X
ln
S
(r 2 T t
/
2)(T
t))
N(d
2)
Since T :
fT
K
0
S
1 T
X1, ST 2
X2
else
and
p(ST X ) N(d2 )
Where
E[ fT ] K
S
1 T
X1, ST 2
X22 )
K[P(ST1 X1) *P(ST 2 X 2 ) ] K[N(d21) * N(d22 )]
(a) The delivery price K in the contract is $44.21. The value of the forward contract after six months is given:
f S Ker(T t) 45 44.21e0.10.5 2.95
The forward price, F Ser(T t) 45e0.10.5 47..31
七 Consider a portfolio that is delta neutral, with a gamma of -5,000 and a vega of -8,000. Suppose that a traded option has a gamma of 0.5, a vega of 2.0, and a delta of 0.6.
T:
fT
K
0
S
1 T
X1, ST 2
X2
else
Solution: Since ln ST ~ N ln S (r 2 / 2() T t), 2 (T t)
p(ST X ) p(lnST ln X ) 1 p(lnST ln X )
1 N (ln X ln S (r 2 / 2)(T t)) T t
ST n ? 3) The varaince of ST is D(ST ) S e (e 2 2 (Tt) 2 (Tt) 1) . What is the variance of ST n ? 4) Using risk-neutral valuation to value the derivative, whose payoff at maturity is
p Xer(T t) N (d2 ) Seq(T t) N (d1 )
d1
ln(S
/
X
)
(r
q T
t
2
/
2)(T
t)
d2 d1 T t
1).What is the price of a European call option on a non-dividend-paying stock when the stock price is $69, the strike price is $70, the risk-free interest rate is 5% per annum, the volatility is 35% per annum, and the time to maturity is six months?
2). Suppose the current value of the index is 500, continuous dividend yields of index is 4% per annum, the risk-free interest rate is 6% per annum . if the price of three-month European index call option with exercise price 490is $20, What is the price of a three-month European index put option with exercise price 490?
四、基于同一股票的看跌期权有相同的到期日.执行价格为$70、$65 和$60,市场价格分为$5、 $3 和$2. 如何构造蝶式差价期权.请用一个表格说明这种策略带来的盈利性.股票价格在什么 范围时,蝶式差价期权将导致损失?
五、 基于同一股票的有相同的到期日敲定价为 $70 的期权市场价格为 $4. 敲 定价$65 的看跌期权的市场价格为 $6。解释如何构造底部宽跨式期权.请用一个 表格说明这种策略带来的盈利性.股票价格在什么范围时,宽跨式期权将导致损 失?
Another traded option with a gamma of 0.8, a vega of 1.2, and a delta of 0.5. What position in the traded two call options and in the underlying asset would make the portfolio gamma ,vega and delta neutral? Solution: If , w1 ,w2 , ,w3 are the amounts of the two traded options and underlying asset included in the portfolio, we require that
by put-call parity 3) What is the price of a European futures put option:current futures price is $19, the strike price is $20, the risk-free interest rate is 12% per annum, the volatility is 20% per annum, and the time to maturity is five months? (保留 2 位小数)
