最新流体力学英文版课后习题答案
流体力学试题(英文版)

第 5 页
西安交通大学本科生课程考试试题标准答案与评分标准(A)
课程名称:
流体力学 (英文)
课时:
64
考试时间: 2007
年 1 月 23 日
Part Ⅰ Concepts
1. Fill in the blank (10×1.5)
①; Continuum ② surface , body; ③ Lagrangian, Euler; ④ gage, absolute; ⑤ compression, increase; ⑥ longer-thiner; ⑦ inviscid; ⑧ 2300 or 2100, diameter; ⑨ velocity; ⑩ major, minor 说明:1)若用中文回答,答案正确不扣分 2)英文单词不唯一,酌情判分
∑Q = ∑Q
in
out
)
6. Suppose that mathematical function
f ( x, y )=2 x 2 + xy 2 y 2
is
stream function for a tow-dimensional flow field,please solve the following problems (1) Is this flow filed potential or irrotational flow (势流或无旋流动)? (2) If fluid density ρ = 1.12kg/m3 and at point (1m,-2m) flow pressure is 4800pa,try to obtain the pressure at point (-2m,1m). (15)
2. Expressions and physical significance
流体力学与传热习题参考解答(英文).

1. Water is pumped at a constant velocity 1m/s from large reservoir resting on the floor to the open top of an absorption tower. The point of discharge is 4 meter above the floor, and the friction losses from the reservoir to the tower amount to 30 J/kg. At what height in the reservoir must the water level be kept if the pump can develop only60 J/kg?2222112f 1U P U P w=Z g+h (Z g+)22ρρ++-+ U 1=0 12P =P 10Z = W=60j/kg f h 30/kg =2U =1m/s 2(60300.5)/g 3m Z =--=21Z Z Z 431m ∆=-=-=2. The fluid (density 1200 kg/m 3 ) is pumped at a constant rate 20 m 3 /h from the large reservoir to the evaporator. The pressure above the reservoir maintains atmosphere pressure and the pressure of the evaporator keeps 200 mmHg (vacuum). The distance between the level of liquid in the reservoir and the exit of evaporator is 15 meter and frictional loss in the pipe is 120 J/kg not including the exit of evaporator, what is the pump effective work and power if the diameter of pipe is 60 mm?22112212f U U Z g+W Z g+h 22ρρP P ++=++ 10P = 5422200P x1.013x10 2.67x10N /m 760=-=- 31200Kg /m ρ= 1U 0= f h 120J /kg =22V 20U 1.97m /s A 3600*4006===π/*. 1Z 0= 2Z 15= 422.67x101.97W 15x9.81120246.88J /kg 12002=-+++= N W Q 246.88x1200x20/3600=1646W ρ==3. Water comes out of the pipe (Φ108x4 mm), as shown in Fig. The friction loss of the pipeline which does not cover the loss at the exit of pipe can be calculated by the following equation:h f =6.5U 2where U is the velocity in the pipe, finda. water velocity at section A-A'.b. water flow rate, in m 3 /h.22112212f U U Z g+Z g+h 22ρρP P +=++ 1U 0= 12P =P 1Z 6m = 2Z 0=2f h 6.5U = 22U 6x9.81 6.5U 2=+ U 2.9m/s = 23V=UA=2.94x01x360082m /h =π/.4. Water passes through the variable pipe. The velocity in the small pipe is 2.5 m/s. The vertical glass tubes are inserted respectively at the section A and B to measure the pressure (see fig.) If the friction loss between two section is 15 J/kg, what is the water column difference between two glass tubes? By the way, draw the relative liquid column height of two tubes in the Fig.a ab b U A U A = 2b U 2.5*(33/47)1.23m /s == 22a a b b a b f U U Z g+Z g+h 22ρρP P +=++ a b Z =Z 22a b b a f U U h 22ρρP P -=-+221.23/2 2.5/21512.63=-+= a b P P R g ρ-=∆ 3312.63R=1.29x10m 9.8x10-∆=5. A centrifugal pump takes brine (density 1180 kg/m 3 , viscosity 1.2 cp) from the bottom of a supply tankand delivers it into another tank. The line between the tanks is 300 m of 25 mm diameter pipe (inner diameter). The flow rate is 2 m 3 /h. In this line, there are two gate valves, four elbows (90o ) and one return bend, what is the friction loss if the roughness of pipe is 0.025 mm?22f fst flocal U U h h h 4f k d 22l ∑=+=+∑ 31180kg /m ρ= 300m, d=0.025m l =3-3v 2m /h =1.2cp=1.2x10Pa.s μ=k=0.025mm k/d=0.025/25=0.001c l r k =0.4 k =1 k =2x0.07=0.14el re k 4x0.75 3 k 1.5-2.2===2u v /A 2/(3600x /4x0.025)1.13m /s π===4u d Re 2.78x10ρμ== f 0.063= 2f 2h 4x0.0063x300/0.025x1.13/2+(0.4+1+2x0.07+4x0.7+1.5)x1.13/2 =197.86J/kg∑=6. The orifice meter (diameter of orifice 0.0001 m) is installed for measuring the flow rate. The indicating liquid of orifice is mercury if U shape pressure gauge reading is 0.6 meter and orifice coefficient can be takenas 0.61, what is the flow rate of water?o u c =20o 0V u s 0.61x /4x0.0001π==835.8x10m /s -=7. Water flows through a pipe with a diameter di 100 mm as shown in figure.a. when the valve is closed, R is 600 mm and h equals 1500 mm. While the valve opens partially, R=400 mm and h=1400 mm, f=0.00625 (Finning factor) and k c =0.5 (contraction coefficient), what is the flow rate of water, in m 3 /h?b. If the valve opens fully, what is the pressure of section 2-2', in N/m 2 ? The equivalent length of the valve is1.5 m and the Fanning factor f keeps the same?(ρH2O =1000kg/m 3, ρHg =13600kg/m 3)(1) the valve opens partially ,for selection 1-1’ and 2-2’ , we have 22112212f 1-2u u gZ gZ h 22ρρP P ++=+++ 2212Hg H o 0 g(R h)39630N/m ρρP =P =-= 2212f1-2c u u u 0 Z =0 h 4f +k 2.13u d 22===l We can get Z1 from the valve closed21Hg H O h=1.5m R=0.6m Z gR/h 6.66m ρρ=-=229.81x6.66u /2 2.13u 39630/1000=++23h u=3.13m/s V 3600x /4x0.1x3.1388.5m /h π==(2) when the valve opens fully, for section 1-1’ and 3-3’, we have 22331113f1-3u u gZ gZ h 22ρρP P ++=+++ 311Z 0 Z 6.66m u =0== 22e f1-3c u 3.1.5h (4f k )(4x0.00625x +0.5) 4.81u d 20.01l l ++=+== 229.81x6.66u /2 4.81u =+ u 3.51m/s =For section 1-1’ and 2-2’22112212f1-2u u gZ gZ h 22ρρP P ++=+++ 112120 Z 6.66 Z 0 u 0 u 3.51P ===== 22f1-2c l u h (4f k )(4x0.00625x15/0.10.5)3.51/226.2J /kg d 2=+=+= 22229.81x6.66 3.15/226.2N 32970mρP =++P =8. The rotameter is installed to measure the water flow rate, as shown in figure. If the total length including equivalent length of pipeline A is 10 m and the reading of rotameter is 2.72 m 3 /h, what is the flow rate for pipeline B? (f A =0.0075, f B =0.0045)For parallel pipe line fA fB total A B22A fA A 2A h h V V +V u (l+le) 2.72h 4f 4x0.0075x10/0.053/2()d 23600x /4x0.053π∑=∑=∑∑== 0.333J /kg = 22B fB B B B 23B B B B u (l+le)h 4f 4x0.0045x2/0.3/2xu 0.333d 2u 2.36m /s V =u A 2.36x /4x0.23600m /h π∑∑======10. A flat furnace wall is constructed of 120 mm layer of sil-o-cel brick, with a thermal conductivity 0.08 w/(m o C), backed by a 150 mm of common brick, of conductivity 0.8 w/(m o C), the temperature of inner face of the wall is 1400 o , and that of the outer face is 200o C.a. What is the heat loss through the wall in w per square meter.b. To reduce the heat loss to 600 w/m 2 by adding a layer of cork with k 0.2 w/(m o C) on the outside of common brick, how many meters of cork are requied? a. 2Q t 1400200711N /m 11L R 0.080.80.120.15∑∆-===∑+ b. 600=(1400-200)/(0.12/0.08+0.15/0.8+x/0.2)x=0.0625m13. Air at the normal pressure passes through the pipe (d i 20 mm) and is heated from 20o C to 100o C. What is the film heat transfer coefficient between the air and pipe wall if the average velocity of air is 10 m/s? The properties of air at 60 o C are as follows:density 1.06 kg/m 3 , viscosity 0.02 cp, conductivity 0.0289 w/(m o C), and heat capacity 1 kJ/kg-K443u d 10x0.02x1.06 Re=1.06x10100.02x10ρμ-==>12T +T 20100T=6022+==℃ 0.141ωμμ⎛⎫= ⎪⎝⎭10000.020.0010.6920.0289p c x x k μ==Pr= ()()0.81/3081/34Nu 0027Re Pr 0.027x 1.06x10x 0.69239.66==.=. ()2i i i h d 39.66 h 39.66x0.0289/0.02=57.22w/m .k k ==14. A hot fluid with a mass flow rate 2250 kg/h passes through a ∅25x2.5 mm tube. The physical properties of fluid are as follows:k=0.5 w/(m o C), C p =4 kJ/kg-K, viscosity 10-3 N-s/m 2 , density 1000 kg/m 3 Find:a. Heat transfer film coefficient h i , in w/(m 2 -K).b. If the flow rate decreases to 1125 kg/h and other conditions are the same, what is the h i ?c. If the diameter of tube (inside diameter) decreases to 10 mm, and the velocity u keeps the same as that of case a, calculate h i .d. When the average temperature of fluid and quantity of heat flow per meter of tube are 40 o C and 400 w/m, respectively, what is the average temperature of pipe wall for case a?e. From this problem, in order to increase the heat transfer film coefficient and enhance heat transfer, what kinds of methods can you use and which is better, explain?Hint: for laminar flow, Nu=1.86[Re Pr]1/3for turbulent flow Nu=0.023Re 0.8 Pr 1/3 (1) 444N 2250x4u d Gd d 3600x x0.02Re 3.98x10100.001ρππμμμ=====> ()()1/30.8081/3424Nu 0023Re Pr 0.023x 3.98x10220.10.5Nuk 220.1x0.5hi 5500w /m k d 0.02⎛⎫== ⎪⎝⎭===.=. (2) 12w 2w = 4421Re Re /2=2x1010=> 0.80.82211Nu Re 0.5Nu Re ⎛⎫== ⎪⎝⎭ 0.8i2i1h 0.5h = ()0.82i2h 5500x0.53159w /m k == (3) 44333u d 2000x0.01Re 2x10100.001ρμ===> 0.81/3Nu 0.023Re Pr = ()2hi=6347w/m k(4)i i w w Q=h A (t-t )=400=500x2x0.02(t-t )πw t=40t 39.41=℃ ℃(5) there methods : increase u or hi or decrease dThe first is better15. In a double pipe exchange (Φ23x2 mm), the cold fluid (Cp=1 kJ/kg, flow rate 500 kg/h) passes throughthe pipe and the hot fluid goes through the outside. The inlet and outlet temperatures of cold fluid are 20 and 80 o , and the inlet and outlet temperatures of hot fluid are 150 and 90o , respectively. The h i (film coefficient inside pipe) is 700 w/(m 2 o C)and overall heat transfer coefficient U o (based on the outside surface of pipe) is 300w/(m 2 o C), respectively. If the heat loss is ignored and the conductivity of pipe wall (steel) is taken as 45 w/(m o C), find:(1) heat transfer film coefficient outside the pipe h o ?(2) the pipe length required for counter flow, in m?(3) what is the pipe length required if the heating medium changes to saturated vapor(140 o C) and it condenses to saturated liquid and other conditions keep unchanged?(4) When the exchanger is used for a year, it is found that it cannot meet the need of production (the outlet temperature of cold fluid cannot reach 80 o C), explain why? (a) 0m o 0i i m d l d 111230.002x23h Vo h d kd 300700x1945x21⎛⎫=-+=-- ⎪⎝⎭ 1/h0=1/U0-(do/hidi+bdo/kdm)=1/300-23/700*19-0.002*23/45*21()20h 642.9w/m k =12t +t LMTD=702∆∆℃= Q=UoAo ∆Tm=mcCp(Tcb-Tca) 300*2π*0.023*70L=500/3600*1000*(80-20)L=5.4m(c) 8020LMTD=86.514020ln 14080-=--℃ 1122L t 70/86.5L t ∆==∆ 2L 0.81L1 4.4m == (d) scale is formed on the outside ,V 0 is decreased16. Water flows turbulently in the pipe of Φ25x2.5 mm shell tube exchanger. When the velocity of water u is 1 m/s, overall heat transfer coefficient Uo (based on the outer surface area of pipe) is 2115 w/(m 2 o C). If the u becomes 1.5 m/s and other conditions keep unchanged, Uo is 2660 w/( m 2 o C ). What is the film coefficient ho outside the pipe? (Heat resistances of pipe wall and scale are ignored)o i h h Uo 111+= (1) oi o h h U 1'1'1+= (2) (1)-(2)= 0.80.80.80.81211111121152660u C u C 1C 1.5C-=-=- C=2859 io h Uo h 111-= ho=8127W/(m2K)17. Water and oil pass parallelly through an exchanger which is 1 m long. The inlet and outlet temperatures of water are 15 and 40 o C, and those of oil are 150 and 100 o C, respectively. If the outlet temperature of oil decreases to 80 o C, and the flow rates and physical properties and inlet temperatures of water and oil maintain the same, what is the pipe length of new exchanger? (Heat loss and pipe wall resistance are neglected) ()()h h 12c c 21m Q W C T -T W C t t VA t ==-=∆()()h h 12c c 21W C T -T 'W C t 't =-2150100401515080t 15--=-- 2t 50=℃ 212m1112m2L T T 't 1508092.51.85L T T t 15010069.8-∆-===-∆- 2m1m2L 1.85m L1=1m t 92.5 t 69.8=∆=∆=18. Air which passes through the pipe in turbulent flow is heated from 20 to 80 o C. The saturated vapor at 116.3 o C condenses to saturated water outside the pipe. If air flow rate increases to 120% of the origin and inlet and outlet temperatures of air stay constant, what kind of method can you employ in order to do that? (Heat resistance of pipe wall and scale can be ignored))(111ca cb pc c m i i T T C m T A h -=∆=1Q)'(2212ca cb pc c m i T T C m T A h -=∆=2Q 128.012112i22.12.1h m m c c m i m T T m m T h T ∆∆===∆∆ )803.116/()203.116ln(20801---=∆m T )80/()20ln(20802---=∆h h m T T T Th=118.5oC19. Water flows through the pipe of a Φ25x2.5 mm shell-tube exchanger from 20 to 50 o C. The hot fluid (C p1.9 kJ/kg o C, flow rate 1.25 kg/s) goes along the shell and the temperatures change from 80 to 30 o C. Film coefficients of water and hot fluid are 0.85kw/(m 2 o C) and 1.7 kw/(m 2 o C). What is the overall heat transfer coefficient Uo and heat transfer area if the scale resistance can be ignored? (the conductivity of steel is 45w/(m o C).W=1.25Kg/s Cp=1.9Kj/kg ℃()()2h p 12Q W C T T 1.25x1.9x 80-30119Kw =-==m 3010t 30ln 10-∆= ()200m 00i i m 1V 472w/m k d l d 1h h d kd ++==32i 0m Q 119x10A 13.9m V t 472x18.2===∆20. A spherical particle (density 2650 kg/m 3) settles freely in air at 20 o C (density of air 1.205 kg/m 3 , viscosity 1x10-5 Pa.s). Calculate the maximum diameter of particle if the settle obeys the Stoke s’ Law?Re ≤1 ()2p t p D g U 18D ρρμμρP -== ()23p 18D g μρρρP =- ()1/3-10p 18x10D 1.205x9.81x 2650-1.205⎛⎫= ⎪ ⎪⎝⎭=3.85x10-521. A filter press(A=0.1 m 2 ) is used for filtering slurry. The vacuum inside the filter is 500 mm Hg. One liter filtrate can be got after filtering of 5 min and 0.6 more liter filtrate is obtained after 5 more min. How much filtrate will be got after filtering of 5 more min?for filter press 22e V 2VV =KA θ+5 min 22e 12V 0.1x5K +=(1)10min 22e 1.62x1.6V 0.1x10K +=(2)From (1) (2),we can see Ve=0.7 K=4815 min 22V 2x0.7V=48x0.1x15+ V=2.07m 3/h22. The following data are obtained for a filter press (A=0.0093 m 2) in a lab.------------------------------------------------------------------------------------------------pressure difference (kg f /cm 2 ) filtering time (s) filtrate volume (m 3 )1.05 502.27⨯10-3660 9.10⨯10-33.50 17.1 2.27⨯10-3233 9.10⨯10-3Find1) filtering constant K, q e , t e at pressure difference 1.05 kg f /cm 2 ?2) if the frame of filter is filled with the cake at 660 s, what is the end filtering rate (dV/dt)E at P 1.05 kg f /cm 2 ?3) compressible constant of cake s?For p=1.05Kg/cm 22e 2e 2e q 2qq K 0.002270.0002272x q 50K 0.00930.000930.000910.000912x q 660K 0.000930.00093θ+=⎛⎫+= ⎪⎝⎭⎛⎫+= ⎪⎝⎭We can see K=0.015 qe=0.026For p=3.5Kg/cm 21-s K=2k ∆P 1-s K'=2k '∆P 1s K 'K '-∆P ⎛⎫= ⎪∆P ⎝⎭ ()2E e V KA 2V+V d d θ⎛⎫= ⎪⎝⎭23. A slurry is filtered by a 0.1 m 2 filter press at constant pressure if the cake is incompressible. The filter basic equation is as follows:(q+10)2 = 250(t+ 0.4)where q---l/m 2 t----minfind (1) how much filtrate is got after 249.6 min?(2) if the pressure difference is double and the resistance of cake is constant, how much filtrate can be obtained after 249.6 min? (cake is imcompressible)(1)let θ=249.6 ()()2q+10250x 249.60.4=+ q=240 V=qA=240*0.1=24(2) K 2k =∆P K'2k '=∆P'2∆P =∆P K'2K 500== ()()2q'+10500x 249.60.4=+ q ’=343.6 v=34.36。
流体力学第二版课后习题答案解析

第一章习题答案选择题(单选题)1.1 按连续介质的概念,流体质点是指:(d )(a )流体的分子;(b )流体内的固体颗粒;(c )几何的点;(d )几何尺寸同流动空间相比是极小量,又含有大量分子的微元体。
1.2 作用于流体的质量力包括:(c )(a )压力;(b )摩擦阻力;(c )重力;(d )表面张力。
1.3 单位质量力的国际单位是:(d )(a )N ;(b )Pa ;(c )kg N /;(d )2/s m 。
1.4 与牛顿内摩擦定律直接有关的因素是:(b )(a )剪应力和压强;(b )剪应力和剪应变率;(c )剪应力和剪应变;(d )剪应力和流速。
1.5 水的动力黏度μ随温度的升高:(b )(a )增大;(b )减小;(c )不变;(d )不定。
1.6 流体运动黏度ν的国际单位是:(a )(a )2/s m ;(b )2/m N ;(c )m kg /;(d )2/m s N ⋅。
1.7 无黏性流体的特征是:(c )(a )黏度是常数;(b )不可压缩;(c )无黏性;(d )符合RT p=ρ。
1.8 当水的压强增加1个大气压时,水的密度增大约为:(a )(a )1/20000;(b )1/10000;(c )1/4000;(d )1/2000。
1.9 水的密度为10003kg/m ,2L 水的质量和重量是多少? 解:10000.0022m V ρ==⨯=(kg )29.80719.614G mg ==⨯=(N )答:2L 水的质量是2kg ,重量是19.614N 。
1.10 体积为0.53m 的油料,重量为4410N ,试求该油料的密度是多少? 解:44109.807899.3580.5m G g V V ρ====(kg/m 3) 答:该油料的密度是899.358kg/m 3。
1.11某液体的动力黏度为0.005Pa s ⋅,其密度为8503/kg m ,试求其运动黏度。
流体力学第1、2、3、4章课后习题答案

第一章习题答案选择题 (单选题)1.1按连续介质的概念,流体质点是指:( d )( a )流体的分子; ( b )流体内的固体颗粒; ( c )几何的点;( d )几何尺寸同流动空间相比是极小量,又含有大量分子的微元体。
1.2作用于流体的质量力包括: ( c )( a )压力;(b )摩擦阻力;( c )重力;( d )表面张力。
1.3 单位质量力的国际单位是: ( d )( a ) N ;( b ) Pa ;( c ) N / kg ;(d ) m / s 2 。
1.4与牛顿内摩擦定律直接有关的因素是:( b )( a )剪应力和压强; ( b )剪应力和剪应变率; ( c )剪应力和剪应变; ( d )剪应力和流速。
1.5 水的动力黏度 μ 随温度的升高: ( b )( a )增大;(b )减小;( c )不变;( d )不定。
1.6 流体运动黏度的国际单位是: ( a )222( a )) N / m ;( );( ) N s/ m 。
bc kg / m d1.7 无黏性流体的特征是: ( c )( a )黏度是常数; ( b )不可压缩;(c )无黏性;( d )符合pRT 。
1.8 当水的压强增加 1 个大气压时,水的密度增大约为:( a )( a ) 1/20000;( b ) 1/10000;( c ) 1/4000 ;( d ) 1/2000。
1.9 水的密度为 1000 kg/m 3 ,2L 水的质量和重量是多少?解:m V 1000 ( kg )0. 002Gmg2 9.80719.614 ( N )答: 2L 水的质量是 2 kg ,重量是 19.614N 。
1.10 体积为 0.5 m 3的油料,重量为 4410N ,试求该油料的密度是多少?解:m G g 4 4 1 0 9 . 8 0879 9 . 3 (58kg/m 3)V V0 . 5答:该油料的密度是899.358 kg/m 3。
流体力学第二版课后答案完整版

第一章习题答案选择题(单选题)1.1 按连续介质的概念,流体质点是指:(d )(a )流体的分子;(b )流体内的固体颗粒;(c )几何的点;(d )几何尺寸同流动空间相比是极小量,又含有大量分子的微元体。
1.2 作用于流体的质量力包括:(c )(a )压力;(b )摩擦阻力;(c )重力;(d )表面张力。
1.3 单位质量力的国际单位是:(d )(a )N ;(b )Pa ;(c )kg N /;(d )2/s m 。
1.4 与牛顿内摩擦定律直接有关的因素是:(b )(a )剪应力和压强;(b )剪应力和剪应变率;(c )剪应力和剪应变;(d )剪应力和流速。
1.5 水的动力黏度μ随温度的升高:(b )(a )增大;(b )减小;(c )不变;(d )不定。
1.6 流体运动黏度ν的国际单位是:(a )(a )2/s m ;(b )2/m N ;(c )m kg /;(d )2/m s N ⋅。
1.7 无黏性流体的特征是:(c )(a )黏度是常数;(b )不可压缩;(c )无黏性;(d )符合RT p=ρ。
1.8 当水的压强增加1个大气压时,水的密度增大约为:(a )(a )1/20000;(b )1/10000;(c )1/4000;(d )1/2000。
1.9 水的密度为10003kg/m ,2L 水的质量和重量是多少? 解:10000.0022m V ρ==⨯=(kg )29.80719.614G mg ==⨯=(N )答:2L 水的质量是2kg ,重量是19.614N 。
1.10 体积为0.53m 的油料,重量为4410N ,试求该油料的密度是多少? 解:44109.807899.3580.5m G g V V ρ====(kg/m 3) 答:该油料的密度是899.358kg/m 3。
1.11 某液体的动力黏度为0.005Pa s ⋅,其密度为8503/kg m ,试求其运动黏度。
流体力学与传热习题参考解答(英文)(完整资料).doc

【最新整理,下载后即可编辑】1. Water is pumped at a constant velocity 1m/s from large reservoir resting on the floor to the open top of an absorption tower. The point of discharge is 4 meter above the floor, and the friction losses from the reservoir to the tower amount to 30 J/kg. At what height in the reservoir must the water level be kept if the pump can develop only60 J/kg?2222112f 1U P U P w=Z g+h (Z g+)22ρρ++-+U 1=012P =P10Z =W=60j/kg f h 30/kg = 2U =1m/s 2(60300.5)/g 3m Z =--= 21Z Z Z 431m ∆=-=-=2. The fluid (density 1200 kg/m 3 ) is pumped at a constant rate 20 m 3 /h from the large reservoir to the evaporator. The pressure above the reservoir maintains atmosphere pressure and the pressure of the evaporator keeps 200 mmHg (vacuum). The distance between the level of liquid in the reservoir and the exit of evaporator is 15 meter and frictional loss in the pipe is 120 J/kg not including the exit of evaporator, what is the pump effective work and power if the diameter of pipe is 60 mm?22112212f U UZ g+W Z g+h 22ρρP P ++=++10P =5422200P x1.013x10 2.67x10N /m 760=-=- 31200Kg /m ρ= 1U 0= f h 120J /kg =22V 20U 1.97m /s A 3600*4006===π/*.1Z 0=2Z 15=422.67x101.97W 15x9.81120246.88J /kg 12002=-+++=N W Q 246.88x1200x20/3600=1646W ρ==3. Water comes out of the pipe (Φ108x4 mm), as shown in Fig. The friction loss of the pipeline which does not cover the loss at the exit of pipe can be calculated by the following equation: h f =6.5U 2where U is the velocity in the pipe, find a. water velocity at section A-A'. b. water flow rate, in m 3 /h.22112212f U UZ g+Z g+h 22ρρP P +=++1U 0=12P =P1Z 6m =2Z 0=2f h 6.5U=22U 6x9.81 6.5U 2=+U 2.9m/s = 23V=UA=2.94x01x360082m /h =π/.4. Water passes through the variable pipe. The velocity in the small pipe is 2.5 m/s. The vertical glass tubes are inserted respectively at the section A and B to measure thepressure (see fig.) If the friction loss between two section is 15 J/kg, what is the water column difference between two glass tubes? By the way, draw the relative liquid column height of two tubes in the Fig.a ab b U A U A =2b U 2.5*(33/47)1.23m /s ==22aa b b a b f U U Z g+Z g+h 22ρρP P +=++a b Z =Z22abb a f U U h 22ρρP P -=-+221.23/2 2.5/21512.63=-+= a b P P R g ρ-=∆ 3312.63R=1.29x10m 9.8x10-∆=5. A centrifugal pump takes brine (density 1180 kg/m 3 , viscosity 1.2 cp) from the bottom of a supply tank and delivers it into another tank. The line between the tanks is 300 m of 25 mm diameter pipe (inner diameter). The flow rate is 2 m 3 /h. In this line, there are two gate valves, four elbows (90o ) and one return bend, what is the friction loss if the roughness of pipe is 0.025 mm?22f fst flocalU U h h h 4f k d 22l ∑=+=+∑31180kg /m ρ=300m, d=0.025m l =3-3v 2m /h =1.2cp=1.2x10Pa.s μ=k=0.025mm k/d=0.025/25=0.001c l r k =0.4 k =1 k =2x0.07=0.14 el re k 4x0.75 3 k 1.5-2.2===2u v /A 2/(3600x /4x0.025)1.13m /s π===4u dRe 2.78x10ρμ==f 0.063=2f 2h 4x0.0063x300/0.025x1.13/2+(0.4+1+2x0.07+4x0.7+1.5)x1.13/2 =197.86J/kg∑=6. The orifice meter (diameter of orifice 0.0001 m) is installed for measuring the flow rate. The indicating liquid of orifice is mercury if U shape pressure gauge reading is 0.6 meter and orifice coefficient can be taken as 0.61, what is the flow rate of water?0o o2gR()u c ρρρ-=20o 02gx0.6x(136001000)V u s 0.61x /4x0.0001x1000π-==835.8x10m /s -=7. Water flows through a pipe with a diameter di 100 mm as shown in figure.a. when the valve is closed, R is 600 mm and h equals 1500 mm. While the valve opens partially, R=400 mm and h=1400 mm, f=0.00625 (Finning factor) and k c =0.5 (contraction coefficient), what is the flow rate of water, in m 3 /h?b. If the valve opens fully, what is the pressure of section 2-2', in N/m 2 ? The equivalent length of the valve is 1.5 m and the Fanning factor f keeps the same?(ρH2O =1000kg/m 3, ρHg =13600kg/m 3)(1) the valve opens partially ,for selection 1-1’ and 2-2’ , we have22112212f 1-2u u gZ gZ h 22ρρP P ++=+++2212Hg H o 0 g(R h)39630N/m ρρP =P =-=2212f1-2c u u u 0 Z =0 h 4f +k 2.13ud 22===lWe can get Z1 from the valve closed21Hg H O h=1.5m R=0.6m Z gR/h 6.66m ρρ=-=229.81x6.66u /2 2.13u 39630/1000=++23h u=3.13m/s V 3600x /4x0.1x3.1388.5m /h π==(2) when the valve opens fully, for section 1-1’ and 3-3’, we have 22331113f1-3u u gZ gZ h 22ρρP P ++=+++311Z 0 Z 6.66m u =0==22e f1-3c u 3.1.5h (4f k )(4x0.00625x +0.5) 4.81ud 20.01l l ++=+==229.81x6.66u /2 4.81u =+ u 3.51m/s =For section 1-1’ and 2-2’22112212f1-2u u gZ gZ h 22ρρP P ++=+++112120 Z 6.66 Z 0 u 0 u 3.51P =====22f1-2c l u h (4f k )(4x0.00625x15/0.10.5)3.51/226.2J /kg d 2=+=+=22229.81x6.66 3.15/226.2N 32970m ρP=++P =8. The rotameter is installed to measure the water flow rate, as shown in figure. If the total length including equivalent length of pipeline A is 10 m and the reading of rotameter is 2.72 m 3 /h, what is the flow rate for pipeline B? (f A =0.0075, f B =0.0045)For parallel pipe linefA fB total A B 22A fAA 2A h h V V +V u (l+le) 2.72h 4f 4x0.0075x10/0.053/2()d 23600x /4x0.053π∑=∑=∑∑== 0.333J /kg =22B fB B B B 23B B B B u (l+le)h 4f 4x0.0045x2/0.3/2xu 0.333d 2u 2.36m /s V =u A 2.36x /4x0.23600m /hπ∑∑======10. A flat furnace wall is constructed of 120 mm layer of sil-o-cel brick, with a thermal conductivity 0.08 w/(m o C), backed by a 150 mm of common brick, of conductivity 0.8 w/(m o C), the temperature of inner face of the wall is 1400 o , and that of the outer face is 200o C.a. What is the heat loss through the wall in w per square meter.b. To reduce the heat loss to 600 w/m 2 by adding a layer of cork with k 0.2 w/(m o C) on the outside of common brick, how many meters of cork are requied? a.2Q t 1400200711N /m 11L R 0.080.80.120.15∑∆-===∑+b. 600=(1400-200)/(0.12/0.08+0.15/0.8+x/0.2) x=0.0625m13. Air at the normal pressure passes through the pipe (d i 20 mm) and is heated from 20o C to 100o C. What is the film heat transfer coefficient between the air and pipe wall if the average velocity of air is 10 m/s? The properties of air at 60 o C are as follows:density 1.06 kg/m 3 , viscosity 0.02 cp, conductivity 0.0289 w/(m o C), and heat capacity 1 kJ/kg-K443u d10x0.02x1.06 Re=1.06x10100.02x10ρμ-==> 12T +T 20100T=6022+==℃ 0.141ωμμ⎛⎫= ⎪⎝⎭10000.020.0010.6920.0289p c x x k μ==Pr=()()0.81/3081/34Nu 0027Re Pr0.027x 1.06x10x 0.69239.66==.=.()2i ii h d 39.66 h 39.66x0.0289/0.02=57.22w/m .k k==14. A hot fluid with a mass flow rate 2250 kg/h passes through a ∅25x2.5 mm tube. The physical properties of fluid are as follows:k=0.5 w/(m o C), C p =4 kJ/kg-K, viscosity 10-3 N-s/m 2 , density 1000 kg/m 3 Find:a. Heat transfer film coefficient h i , in w/(m 2 -K).b. If the flow rate decreases to 1125 kg/h and other conditions are the same, what is the h i ?c. If the diameter of tube (inside diameter) decreases to 10 mm, and the velocity u keeps the same as that of case a, calculate h i .d. When the average temperature of fluid and quantity of heat flow per meter of tube are 40 o C and 400 w/m, respectively, what is the average temperature of pipe wall for case a?e. From this problem, in order to increase the heat transfer film coefficient and enhance heat transfer, what kinds of methods can you use and which is better, explain?Hint: for laminar flow, Nu=1.86[Re Pr]1/3for turbulent flow Nu=0.023Re 0.8 Pr 1/3 (1)444N 2250x4u d Gd d 3600x x0.02Re 3.98x10100.001ρππμμμ=====>()()1/30.8081/3424Nu 0023Re Pr 0.023x 3.98x10220.10.5Nuk 220.1x0.5hi 5500w /m k d 0.02⎛⎫== ⎪⎝⎭===.=.(2)12w 2w =4421Re Re /2=2x1010=>0.80.82211Nu Re 0.5Nu Re ⎛⎫== ⎪⎝⎭0.8i2i1h 0.5h = ()0.82i2h 5500x0.53159w /m k ==(3) 44333u d 2000x0.01Re 2x10100.001ρμ===>0.81/3Nu 0.023Re Pr =()2hi=6347w/m k(4)i i w w Q=h A (t-t )=400=500x2x0.02(t-t )πw t=40t 39.41=℃ ℃(5) there methods : increase u or hi or decrease d The first is better15. In a double pipe exchange (Φ23x2 mm), the cold fluid (Cp=1 kJ/kg, flow rate 500 kg/h) passes through the pipe and the hot fluid goes through the outside. The inlet and outlet temperatures of cold fluid are 20 and 80 o , and the inlet and outlet temperatures of hot fluid are 150 and 90o , respectively. The h i (film coefficient inside pipe) is 700 w/(m 2 o C)and overall heat transfer coefficient U o (based on the outside surface of pipe) is 300w/(m 2 o C), respectively. If the heat loss is ignored and the conductivity of pipe wall (steel) is taken as 45 w/(m o C), find: (1) heat transfer film coefficient outside the pipe h o ?(2) the pipe length required for counter flow, in m?(3) what is the pipe length required if the heating medium changes to saturatedvapor(140 o C) and it condenses to saturated liquid and other conditions keep unchanged?(4) When the exchanger is used for a year, it is found that it cannot meet the need of production (the outlet temperature of cold fluid cannot reach 80 o C), explain why? (a)0m o 0i i m d l d 111230.002x23h Vo h d kd 300700x1945x21⎛⎫=-+=-- ⎪⎝⎭ 1/h0=1/U0-(do/hidi+bdo/kdm)=1/300-23/700*19-0.002*23/45*21 ()20h 642.9w/m k =12t +t LMTD=702∆∆℃=Q=UoAo ∆Tm=mcCp(Tcb-Tca)300*2π*0.023*70L=500/3600*1000*(80-20)L=5.4m (c) 8020LMTD=86.514020ln14080-=--℃1122L t 70/86.5L t ∆==∆ 2L 0.81L1 4.4m == (d) scale is formed on the outside ,V 0 is decreased16. Water flows turbulently in the pipe of Φ25x2.5 mm shell tube exchanger. When the velocity of water u is 1 m/s, overall heat transfer coefficient Uo (based on the outer surface area of pipe) is 2115 w/(m 2 o C). If the u becomes 1.5 m/s and other conditions keep unchanged, Uo is 2660 w/( m 2 o C ). What is the film coefficient ho outside the pipe? (Heat resistances of pipe wall and scale are ignored)oi h h Uo 111+= (1)o i o h h U 1'1'1+= (2) (1)-(2)=0.80.80.80.81211111121152660u C u C 1C 1.5C-=-=-C=2859 io h Uo h 111-= ho=8127W/(m2K)17. Water and oil pass parallelly through an exchanger which is 1 m long. The inlet andoutlet temperatures of water are 15 and 40 o C, and those of oil are 150 and 100 o C, respectively. If the outlet temperature of oil decreases to 80 o C, and the flow rates and physical properties and inlet temperatures of water and oil maintain the same, what is the pipe length of new exchanger? (Heat loss and pipe wall resistance are neglected) ()()h h 12c c 21m Q W C T -T W C t t VA t ==-=∆ ()()h h 12c c 21W C T -T 'W C t 't =- 2150100401515080t 15--=-- 2t 50=℃212m1112m2L T T 't 1508092.51.85L T T t 15010069.8-∆-===-∆- 2m1m2L 1.85m L1=1m t 92.5 t 69.8=∆=∆=18. Air which passes through the pipe in turbulent flow is heated from 20 to 80 o C. The saturated vapor at 116.3 o C condenses to saturated water outside the pipe. If air flow rate increases to 120% of the origin and inlet and outlet temperatures of air stay constant, what kind of method can you employ in order to do that? (Heat resistance of pipe wall and scale can be ignored))(111ca cb pc c m i i T T C m T A h -=∆=1Q)'(2212ca cb pc c m i T T C m T A h -=∆=2Q 128.012112i22.12.1h m m c c m i m T T m m T h T ∆∆===∆∆ )803.116/()203.116ln(20801---=∆m T)80/()20ln(20802---=∆h h m T T TTh=118.5oC19. Water flows through the pipe of a Φ25x2.5 mm shell-tube exchanger from 20 to 50 o C. The hot fluid (C p 1.9 kJ/kg o C, flow rate 1.25 kg/s) goes along the shell and the temperatures change from 80 to 30 o C. Film coefficients of water and hot fluid are0.85kw/(m 2 o C) and 1.7 kw/(m 2 o C). What is the overall heat transfer coefficient Uo and heat transfer area if the scale resistance can be ignored? (the conductivity of steel is 45w/(m o C).W=1.25Kg/s Cp=1.9Kj/kg ℃()()2h p 12Q W C T T 1.25x1.9x 80-30119Kw =-==m 3010t 30ln 10-∆= ()200m 00i i m 1V 472w/m k d l d 1h h d kd ++==32i 0m Q 119x10A 13.9m V t 472x18.2===∆20. A spherical particle (density 2650 kg/m 3) settles freely in air at 20 o C (density of air 1.205 kg/m 3 , viscosity 1x10-5 Pa.s). Calculate the maximum diameter of particle if the settle obeys the Stoke s’ Law?Re ≤1 ()2p t p D g U 18D ρρμμρP -== ()23p 18D g μρρρP =-()1/3-10p 18x10D 1.205x9.81x 2650-1.205⎛⎫= ⎪ ⎪⎝⎭=3.85x10-521. A filter press(A=0.1 m 2 ) is used for filtering slurry. The vacuum inside the filter is 500 mm Hg. One liter filtrate can be got after filtering of 5 min and 0.6 more liter filtrate is obtained after 5 more min. How much filtrate will be got after filtering of 5 more min?for filter press 22e V 2VV =KA θ+5 min 22e 12V 0.1x5K +=(1)10min 22e 1.62x1.6V 0.1x10K +=(2)From (1) (2),we can see Ve=0.7 K=4815 min 22V 2x0.7V=48x0.1x15+ V=2.07m 3/h22. The following data are obtained for a filter press (A=0.0093 m 2) in a lab.------------------------------------------------------------------------------------------------pressure difference (kg f /cm 2 ) filtering time (s) filtrate volume (m 3 )1.05 502.27⨯10-3660 9.10⨯10-33.50 17.1 2.27⨯10-3233 9.10⨯10-3Find1) filtering constant K, q e , t e at pressure difference 1.05 kg f /cm 2 ?2) if the frame of filter is filled with the cake at 660 s, what is the end filtering rate (dV/dt)E at P 1.05 kg f /cm 2 ?3) compressible constant of cake s?For p=1.05Kg/cm 22e 2e 2e q 2qq K 0.002270.0002272x q 50K 0.00930.000930.000910.000912x q 660K 0.000930.00093θ+=⎛⎫+= ⎪⎝⎭⎛⎫+= ⎪⎝⎭ We can see K=0.015 qe=0.026For p=3.5Kg/cm 21-s K=2k ∆P 1-s K'=2k '∆P1s K 'K '-∆P ⎛⎫= ⎪∆P ⎝⎭ ()2E e V KA 2V+V d d θ⎛⎫= ⎪⎝⎭23. A slurry is filtered by a 0.1 m 2 filter press at constant pressure if the cake is incompressible. The filter basic equation is as follows:(q+10)2 = 250(t+ 0.4)where q---l/m 2 t----minfind (1) how much filtrate is got after 249.6 min?(2) if the pressure difference is double and the resistance of cake is constant, how much filtrate can be obtained after 249.6 min? (cake is imcompressible)(1)let θ=249.6 ()()2q+10250x 249.60.4=+q=240 V=qA=240*0.1=24 (2) K2k=∆P=∆P K'2k'∆P=∆P K'2K500'2==()()2q'+10500x249.60.4=+q’=343.6 v=34.36。
流体力学英文版课后习题问题详解
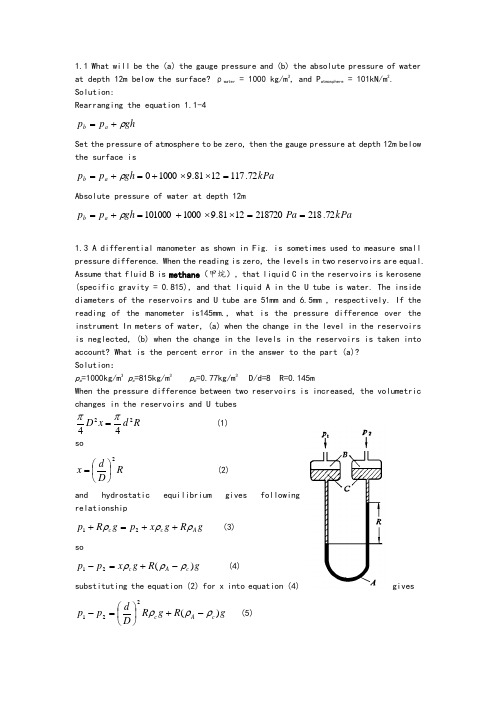
1.1 What will be the (a) the gauge pressure and (b) the absolute pressure of waterat depth 12m below the surface? ρwater = 1000 kg/m 3, and P atmosphere = 101kN/m 2.Solution:Rearranging the equation 1.1-4gh p p a b ρ+=Set the pressure of atmosphere to be zero, then the gauge pressure at depth 12m belowthe surface iskPa gh p p a b 72.1171281.910000=⨯⨯+=+=ρAbsolute pressure of water at depth 12mkPa Pa gh p p a b 72.2182187201281.91000101000==⨯⨯+=+=ρ1.3 A differential manometer as shown in Fig. is sometimes used to measure smallpressure difference. When the reading is zero, the levels in two reservoirs are equal.Assume that fluid B is methane (甲烷), that liquid C in the reservoirs is kerosene(specific gravity = 0.815), and that liquid A in the U tube is water. The insidediameters of the reservoirs and U tube are 51mm and 6.5mm , respectively. If thereading of the manometer is145mm., what is the pressure difference over theinstrument In meters of water, (a) when the change in the level in the reservoirsis neglected, (b) when the change in the levels in the reservoirs is taken intoaccount? What is the percent error in the answer to the part (a)?Solution :p a =1000kg/m 3 p c =815kg/m 3 p b =0.77kg/m 3 D/d=8 R=0.145mWhen the pressure difference between two reservoirs is increased, the volumetric changes in the reservoirs and U tubesR d x D 2244ππ= (1) so R D d x 2⎪⎭⎫ ⎝⎛= (2) and hydrostaticequilibrium gives followingrelationship g R g x p g R p A c c ρρρ++=+21 (3)sog R g x p p c A c )(21ρρρ-+=- (4)substituting the equation (2) for x into equation (4) givesg R g R D d p p c A c )(221ρρρ-+⎪⎭⎫ ⎝⎛=- (5)(a )when the change in the level in the reservoirs is neglected,()Pa g R g R g R D d p p c A c A c 26381.98151000145.0)()(221=⨯-=-≈-+⎪⎭⎫ ⎝⎛=-ρρρρρ(b )when the change in the levels in the reservoirs is taken into account ()Pa g R g R D d g R g R D d p p c A c c A c 8.28181.98151000145.081.9815145.0515.6)()(22221=⨯-+⨯⨯⨯⎪⎭⎫ ⎝⎛=-+⎪⎭⎫ ⎝⎛=-+⎪⎭⎫ ⎝⎛=-ρρρρρρ error=%=7.68.2812638.281- 1.4 There are two U-tube manometers fixed on the fluid bed reactor, as shown in thefigure. The readings of two U-tube manometers are R 1=400mm ,R 2=50mm, respectively.The indicating liquid is mercury. The top of the manometer is filled with the waterto prevent from the mercury vapor diffusing into the air, and the height R 3=50mm.Try to calculate the pressure at point A and B .Solution: There is a gaseous mixture in the U-tube manometer meter. The densitiesof fluids are denoted by Hg O H g ρρρ,,2, respectively. The pressure at point AFigure for problem 1.4is given by hydrostatic equilibriumg R R g R g R p g Hg O H A )(32232+-+=ρρρg ρis small and negligible in comparison with Hg ρand ρH2O , equation above can be simplifiedc A p p ≈=232gR gR Hg O H ρρ+=1000×9.81×0.05+13600×9.81×0.05=7161N/m ²1gR p p p Hg A D B ρ+=≈=7161+13600×9.81×0.4=60527N/m1.5 Water discharges from the reservoir through the drainpipe, which the throat diameter is d. The ratio of D to d equals 1.25. The vertical distance h between the tank A and axis of the drainpipe is 2m. What height H from the centerline of the drainpipe to the water level in reservoir is required for drawing the water from the tank A to the throat of the pipe? Assume thatfluid flow is a potentialflow. The reservoir, tankA and the exit of drainpipeare all open to air.Solution:Bernoulli equation is written between stations 1-1 and 2-2, with station 2-2 being reference plane:2222222111u gz p u gz p ++=++ρρ Where p 1=0, p 2=0, and u 1=0, simplification of the equation1The relationship between the velocity at outlet and velocity u o at throat can be derived by the continuity equation:22⎪⎭⎫ ⎝⎛=⎪⎪⎭⎫ ⎝⎛D d u u o 22⎪⎭⎫ ⎝⎛=d D u u o 2 Bernoulli equation is written between the throat and the station 2-2 3Combining equation 1,2,and 3 givesSolving for HH=1.39m1.6 A liquid with a constant density ρ kg/m 3is flowing at an unknown velocity V 1m/s through a horizontal pipe of cross-sectional area A 1 m 2 at a pressure p 1 N/m 2,and then it passes to a section of the pipe in which the area is reduced graduallyto A 2 m 2 and the pressure is p 2. Assuming no friction losses, calculate the velocities V 1 and V 2 if the pressure difference (p 1 - p 2) is measured. Solution :In Fig1.6, the flow diagram is shown with pressure taps to measure p 1 and p 2. From the mass-balance continuity equation , for constant ρ where ρ1 = ρ2 = ρ,222u Hg =222200u u p =+ρ()===144.281.92100081.910002125.11112442-⨯⨯⨯--⎪⎭⎫ ⎝⎛==ρρg h d D u Hg2112A A V V = For the items in the Bernoulli equation , for a horizontal pipe,z 1=z 2=0 Then Bernoulli equation becomes, after substituting 2112A A V V = for V 2, ρρ22121211212020p A A V p V ++=++ Rearranging,2)1(21212121-=-A A V p p ρ ⎥⎥⎦⎤⎢⎢⎣⎡-⎪⎪⎭⎫ ⎝⎛-12221211A A p p V ρ=Performing the same derivation but in terms of V 2,⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛--21221212A A p p V ρ=1.7 A liquid whose coefficient of viscosity is µ flows below the critical velocity for laminar flow in a circular pipe of diameter d and with mean velocity V . Show that the pressure loss in a length of pipe L p ∆ is 232d V μ. Oil of viscosity 0.05 Pas flows through a pipe of diameter 0.1m with a average velocity of 0.6m/s. Calculate the loss of pressure in a length of 120m.Solution : The average velocity V for a cross section is found by summing up all the velocities over the cross section and dividing by the cross-sectional area1From velocity profile equation for laminar flow2 substituting equation 2 for u into equation 1 and integrating3rearranging equation 3 gives1.8. In a vertical pipe carrying water, pressuregauges are inserted at points A and B where the pipediameters are 0.15m and 0.075m respectively. Thepoint B is 2.5m below A and when the flow rate downthe pipe is 0.02 m 3/s, the pressure at B is 14715N/m 2 greater than that at A. Figure for problem 1.8⎰⎰==R R rdr u R udA A V 020211ππ⎪⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛--=22014R r R L p p u L μ2032D L p p V L μ-=232d V L p μ=∆Pa d VL p 115201.01206.005.0323222=⨯⨯⨯==∆μAssuming the losses in the pipe between A and B can be expressed as g V k 22where V is the velocity at A, find the value of k .If the gauges at A and B are replaced by tubes filled with water and connected to a U-tube containing mercury of relative density 13.6, give a sketch showing how the levels in the two limbs of the U-tube differ and calculate the value of this difference in metres.Solution:d A =0.15m; d B =0.075mz A -z B =l =2.5mQ =0.02 m 3/s,p B -p A =14715 N/m 2s m d QV V d Q A A AA /132.115.0785.002.044222=⨯===ππ s m d QV V d Q B B BB /529.4075.0785.002.044222=⨯===ππWhen the fluid flows down, writing mechanical balance equation222222A B B B A A A V k V g z p V g z p +++=++ρρ 213.1253.4100014715213.181.95.2222k ++=+⨯ k 638.0260.10715.14638.0525.24++=+=k 0.295making the static equilibriumgR g x g l p g R g x p Hg A B ρρρρρ+∆++=+∆+()()mm g g l p p R g H A B 7981.91260081.910005.214715-=⨯⨯⨯-=---=ρρρ1.9.The liquid vertically flows down through the tube fromthe station a to the station b , then horizontally throughthe tube from the station c to the station d, as shown inFigure for problem 1.9figure. Two segments of the tube, both ab and cd ,have the same length, the diameter and roughness.Find:(1)the expressions of gp ab ρ∆, h fab , g p cd ρ∆ and h fcd , respectively. (2)the relationship between readings R 1and R 2 in the U tube.Solution:(1) From Fanning equationandsoFluid flows from station a to station b , mechanical energy conservation giveshence2from station c to station dhence3From static equationp a -p b =R 1(ρˊ-ρ)g -l ρg 4p c -p d =R 2(ρˊ-ρ)g 5Substituting equation 4 in equation 2 ,thentherefore6 22V d l h fab λ=22V d l h fcd λ=fcdfab h h =fab b a h p p +=+ρρlg fab b a h p p =+-lg ρfcd d c h p p +=ρρfcd d c h p p =-ρfab h g l g R =+--'lg 1ρρρρ)(g R h fab ρρρ-'=1Substituting equation 5 in equation 3 ,then7ThusR 1=R 21.10 Water passes through a pipe of diameter d i=0.004 m with the average velocity 0.4 m/s, as shown in Figure.1) What is the pressure drop –∆P when water flows through the pipe length L =2 m, in m H 2O column?2) Find the maximum velocity and point r at which it occurs.3) Find the point r at which the averagevelocity equals the local velocity. 4)if kerosene flows through this pipe ,howdo the variables above change ?(the viscosity and density of Water are0.001 Pas and 1000 kg/m 3,respectively ;andthe viscosity and density of kerosene are0.003 Pas and 800 kg/m 3,respectively )solution:1)1600001.01000004.04.0Re =⨯⨯==μρud from Hagen-Poiseuille equation1600004.0001.024.0323222=⨯⨯⨯==∆d uL P μ m g p h 163.081.910001600=⨯=∆=ρ 2)maximum velocity occurs at the center of pipe, from equation 1.4-19 max 0.5V u =so u max =0.4×2=0.8m3)when u=V=0.4m/s Eq. 1.4-172max 1⎪⎪⎭⎫ ⎝⎛-=w r r u u g R h fcd ρρρ-'=2Figure for problem 1.105.0004.01max2=⎪⎭⎫ ⎝⎛-u V r = m r 00284.071.0004.05.0004.0=⨯== 4) kerosene: 427003.0800004.04.0Re =⨯⨯==μρud Pa p p 4800001.0003.01600=='∆='∆μμ m g p h 611.081.98004800=⨯=''∆='ρ1.12 As shown in the figure, the water level in the reservoir keeps constant. A steel drainpipe (with the inside diameter of 100mm) is connected to the bottom of the reservoir. One arm of the U-tube manometer is connected to the drainpipe at the position 15m away from the bottom of the reservoir, and the other is opened to the air, the U tube is filled with mercury and the left-side arm of the U tube above the mercury is filled with water. The distance between the upstream tap and the outlet of the pipeline is 20m.a) When the gate valve is closed, R=600mm, h=1500mm; when the gate valve is opened partly, R=400mm, h=1400mm. The friction coefficient λ is 0.025, and the loss coefficient of the entrance is 0.5. Calculate the flow rate of water when the gate valve is opened partly. (in m ³/h)b) When the gate valve is widely open, calculate the static pressure at the tap (in gauge pressure, N/m ²). l e /d ≈15 when the gate valve is widely open, and the friction coefficient λ is still 0.025.Solution :(1) When the gate valve is opened partially, the water discharge isSet up Bernoulli equation between the surface of reservoir 1—1’and the sectionof pressure point 2—2’,and take the center of section 2—2’as the referring plane, then∑+++=++21,2222121122—f h p u gZ p u gZ ρρ (a )In the equation 01=p (the gauge pressure)222/396304.181.910004.081.913600m N gh gR p O H Hg =⨯⨯-⨯⨯=-=ρρ 0021=≈Z uWhen the gate valve is fully closed, the height of water level in the reservoircan be related to h (the distance between the center of pipe and the meniscus of left arm of U tube).gR h Z g Hg O H ρρ=+)(12(b )where h=1.5mR=0.6mSubstitute the known variables into equation b2222_1,113.22)5.01.015025.0(2)(66.65.110006.013600V VV K d l h mZ cf =+⨯=+==-⨯=∑λ Substitute the known variables equation a9.81×6.66=2213.21000396302V V ++ the velocity is V =3.13m/sthe flow rate of water isFigure for problem 1.12h m V d V h /5.8813.312.0436004360032=⨯⨯⨯=⨯=ππ2) the pressure of the point where pressure is measured when the gate valve is wide-open.Write mechanical energy balance equation between the stations 1—1’and 3-3´,then∑+++=++31,3233121122—f h p V gZ p V gZ ρρ (c )since m Z 66.61=311300p p u Z =≈= 2223_1,81.4 2]5.0)151.035(025.0[ 2)(V V V K d l l h c e f =++=++=∑λ input the above data into equation c ,9.8122V 81.4266.6+=⨯V the velocity is: V =3.51 m/sWrite mechanical energy balance equation between thestations 1—1’and 2——2’, for the same situation of water level∑+++=++21,2222121122—f h p V gZ p V gZ ρρ(d )since m Z 66.61=2121003.51/0(page pressure Z u u m sp =≈≈=)kg J V K d l hc f /2.26251.3)5.01.015025.0(2)(222_1,=+⨯=+=∑λinput the above data into equation d ,9.81×6.66=2.261000251.322++p the pressure is: 329702=p1.14 Water at 20℃ passes through a steel pipe with an inside diameter of 300mm and 2m long. There is a attached-pipe (Φ60⨯3.5mm) which is parallel with the main pipe. The total length including the equivalent length of all form losses of the attached-pipe is 10m. A rotameter is installed in the branch pipe. When the readingof the rotameter is 2.72m 3/h, try to calculate the flow rate in the main pipe and the total flow rate, respectively. The frictional coefficient of the main pipe and the attached-pipe is 0.018 and 0.03, respectively.Solution : The variables of main pipe are denoted by a subscript1, and branch pipeby subscript 2. The friction loss for parallel pipelines is 2121S S s f f V V V h h+==∑∑The energy loss in the branch pipe is22222222u d l l he f ∑∑+=λ In the equation 03.02=λsm u d ml l e /343.0053.04360072.2053.01022222=⨯⨯===+∑πinput the data into equation c kg J hf /333.02343.0053.01003.022=⨯⨯=∑The energy loss in the main pipe is333.022111121===∑∑u d l h hf f λSo s m u /36.22018.023.0333.01=⨯⨯⨯=The water discharge of main pipe ish m V h /60136.23.043600321=⨯⨯⨯=πTotal water discharge ish m V h /7.60372.26013≈+=1.16 A Venturimeter is used for measuring flow of water along a pipe. The diameter of the Venturi throat is two fifths the diameter of the pipe. The inlet and throat are connected by water filled tubes to a mercury U-tube manometer. The velocity of flow along the pipe is found to be R 5.2 m/s, where R is the manometer reading in metres of mercury. Determine the loss of head between inlet and throat of the Venturi when R is 0.49m. (Relative density of mercury is 13.6). Solution: Writing mechanical energy balance equation between the inlet 1 and throat o for Venturi meterf o o hg z V p g z V p +++=++22121122ρρ 1 rearranging the equation above, and set (z 2-z 1)=xf o oh xg V V p p ++-=-22121ρ2from continuity equation11221125.625V V dd V V o o =⎪⎭⎫ ⎝⎛=⎪⎪⎭⎫ ⎝⎛= 3 substituting equation 3 for V o into equation 2 gives()fff f oh xg R h xg Rh V h xg V V p p ++=++=+=++-=-94.1185.203.1903.19206.3922121211ρ4from the hydrostatic equilibrium for manometerg x g R p p Hg o ρρρ+-=-)(1 5substituting equation 5 for pressure difference into equation 4 obtainsf Hgh xg R gx g R ++=+-94.118)(ρρρρ 6rearranging equation 6Figure for problem 1.16kg J R R R R gR h Hg f /288.267.494.11861.12394.118)(==-=--=ρρρ1.17.Sulphuric acid of specific gravity 1.3 is flowing through a pipe of 50 mm internal diameter. A thin-lipped orifice, 10mm, is fitted in the pipe and the differential pressure shown by a mercury manometer is 10cm. Assuming that the leads to the manometer are filled with the acid, calculate (a)the weight of acid flowing per second, and (b) the approximate friction loss in pressure caused by the orifice.The coefficient of the orifice may be taken as 0.61, the specific gravity of mercuryas 13.6, and the density of water as 1000 kg/m 3Solution:a)2.0501010==D D =⨯-=-=-81.9)130013600(1.0)(21g R p p Hg ρρs kg V D m /268.0130063.201.0442220=⨯⨯⨯==πρπb) approximate pressure drop=⨯-=-=-81.9)130013600(1.0)(21g R p p Hg ρρ12066.3Papressure difference due to increase of velocity in passing through the orificePa D D V V V V p p o8.44882)2.01(63.213002242412222212221=-=⎪⎪⎭⎫⎝⎛-=-=-ρρpressure drop caused by friction lossPa p f 5.75778.44883.12066=-=∆()s m p p D D C V o /63.231.461.056.1861.0130081.9)130013600(1.022.0161.021*******=⨯=≈⨯-⨯-=-⎪⎪⎭⎫ ⎝⎛-=ρ2.1 Water is used to test for the performances of pump. The gauge pressure at the discharge connection is 152 kPa and the reading of vacuum gauge at the suctionconnection of the pump is 24.7 kPa as the flow rate is 26m 3/h. The shaft power is 2.45kw while the centrifugal pump operates at the speed of 2900r/min. If the vertical distance between the suction connection and discharge connection is 0.4m, the diameters of both the suction and discharge line are the same. Calculate the mechanical efficiency of pump and list the performance of the pump under this operating condition. Solution:Write the mechanical energy balance equation between the suction connection and discharge connection2_1,2222121122f H gp g u Z H g p g u Z +++=+++ρρwherem Z Z 4.012=-(Pa 1052.1(Pa 1047.22_1,215241≈=⨯=⨯-=f H u u pressure gauge p pressure gauge p ))total heads of pump is m H 41.1881.9100010247.01052.14.055=⨯⨯+⨯+= efficiency of pump is N N e /=η since kW g QH N e 3.1360081.9100041.18263600=⨯⨯⨯==ρ N=2.45kWThen mechanical efficiency%1.53%10045.23.1=⨯=η The performance of pump isFlow rate ,m ³/h26Total heads ,m 18.41Shaftpower ,kW 2.45Efficiency,% 53.12.2 Water is transportedby a pump from reactor, which has 200 mm Hg vacuum, to the tank, in which the gaugepressure is 0.5 kgf/cm 2, as shown in Fig. The total equivalent length of pipe is 200 m including all local frictional loss. The pipeline is φ57×3.5 mm , the orifice coefficient of C o and orifice diameter d o are 0.62 and 25 mm, respectively. Frictional coefficient λ is 0.025. Calculate: Developed head H of pump, in m (the reading R of U pressure gauge in orifice meter is 168 mm Hg)Solution:Equation(1.6-9)Mass flow rates kg S V m o o /02.21000025.0414.312.42=⨯⨯⨯==ρ 2) Fluid flow through the pipe from the reactor to tank, the Bernoulli equation is as follows for V 1=V 2f H z gp p H +∆+-=ρ12 ∆z=10mPa p 7570710013.17602001081.95.054=⨯⨯+⨯⨯=∆ ∆p/ρg=7.7mThe relation between the hole velocity and velocity of pipeFriction losssoH=7.7+10+5.1=22.8m2.3 . A centrifugal pump is to be used to extract water from a condenser in which the vacuum is 640 mm of mercury, as shown in figure. At the rated discharge, the net positive suction head must be at least 3m above the cavitation vapor pressure of 710mm mercury vacuum.Ifs m Rg D d C V f /12.444.69375.062.01000)100013600(81.9168.025025162.02144000=⨯=-⨯⨯⎪⎭⎫⎝⎛-=-⎪⎭⎫ ⎝⎛-=ρρρ)(s m D d V V /12112.42200=⎪⎭⎫⎝⎛⨯=⎪⎭⎫ ⎝⎛=m g u d l f H f 1.581.92105.0200025.02422=⨯⨯==losses in the suction pipe accounted for a head of 1.5m. What must be the least height of the liquid level in the condenser above the pump inlet? Solution :From an energy balance,WhereP o =760-640=120mmHg P v =760-710=50mmHgUse of the equation will give the minimum height H g as2.4 Sulphuric acid is pumped at 3 kg/s through a 60m length of smooth 25 mm pipe. Calculate the drop in pressure. If the pressure drop falls by one half, what will the new flowrate be ?• Density of acid 1840kg/m 3• Viscosity of acid 25×10-3PasSolution:Velocity of acid in the pipe:sm d m d mpipe of area tional cross flowrate volumetric u /32.3025.01840785.03785.04sec 222=⨯⨯===-=ρπρReynolds number:6109102532.31840025.0Re 3=⨯⨯⨯==-μρud from Fig.1.22 for a smooth pipe when Re=6109, f=0.0085 pressure drop is calculated from equation 1.4-9kg J u d l f ph f /450232.3025.0600085.042422=⨯==∆=ρkPa p 5.8271840450=⨯=∆or friction factor is calculated from equation1.4-25NPSH H gp p H f vo g ---=ρmNPSH H gp p H f vo g 55.335.181.9100081.913600)05.012.0-=--⨯⨯⨯-=---=(ρkgJ u d l u d l f ph f /426232.3025.0606109046.042Re 046.042422.022.02=⨯⨯⨯==∆=--=ρkPa p 84.7831840426=⨯=∆if the pressure drop falls to 783.84/2=391.92kPa8.18.12.12.038.12.12.022.0012.089.1079`2025.060102518401840046.042046.042Re 046.043919202u u u dl u d l p p =⎪⎭⎫ ⎝⎛⨯⨯⨯=⎪⎪⎭⎫ ⎝⎛⨯⨯⨯==∆='∆----ρμρρ=sos m u /27.236.489..1079012.03919208.18.1==⨯=new mass flowrate=0.785d 2u ρ=0.785×0.0252×2.27×1840=2.05kg/s2.4 Sulphuric acid is pumped at 3 kg/s through a 60m length of smooth 25 mm pipe. Calculate the drop in pressure. If the pressure drop falls by one half on assumption that the change of friction factor is negligible, what will the new flowrate be ?Density of acid 1840kg/m 3Viscosity of acid 25×10-3Pa Friction factor 32.0Re500.00056.0+=f for hydraulically smooth pipe Solution:Write energy balance equation:f h gu z g p H g u z g p +++=+++2222222111ρρ gu d l g p h H f 22λρ=∆==342=ρπu ds m d u /32.31840025.014.3124322=⨯⨯=⨯=ρπ 611510251840025.032.3Re 3=⨯⨯⨯=-0087.061155.00056.0Re 500.00056.032.032.0=+=+=f92.4681.9232.3025.0600087.04222=⨯⨯==∆==g u d l g p h H f λρΔp=46.92×1840×9.81=847.0kpa2.6 The fluid is pumped through the horizontal pipe from section A to B with the φ38⨯2.5mm diameter and length of 30 meters, shown as figure. The orifice meter of 16.4mm diameter is used to measure the flow rate. Orifice coefficient C o =0.63.the permanent loss in pressure is 3.5×104N/m 2, the friction coefficient λ=0.024. find:(1) What is the pressure drop along the pipe AB?(2)What is the ratio of power obliterated in pipe AB to total power supplied to thefluid when the shaft work is 500W, 60%efficiency? (The density of fluid is 870kg/m 3)solution :∑+++=+++f AA A A AA h u p g z w u p g z 2222ρρρλρ022p u d l h p p f BA ∆+==-∑247.0334.162=⎪⎭⎫⎝⎛=A A o ()()s m gR C u /5.8870870136006.081.9297.063.02247.01200=-⨯⨯=''--=ρρρ∴u = (16.4/33)2×8.5=2.1m/s∴242/76855105.321.2033.030870024.0m N h p p f B A =⨯+⨯==-∑ρ (2)W u d p Wm 1381.2033.0785.0768554Ne 22=⨯⨯⨯=∆==ρπρ sothe ratio of power obliterated in friction losses in AB to total power supplied to the fluid%%=461006.0500138⨯⨯。
流体力学和传热习题参考解答(英文)

1. Water is pumped at a constant velocity 1m/s from large reservoir resting on the floor to the open top of an absorption tower. The point of discharge is 4 meter above the floor, and the friction losses from the reservoir to the tower amount to 30 J/kg. At what height in the reservoir must the water level be kept if the pump can develop only60 J/kg?2222112f 1U P U P w=Z g+h (Z g+)22ρρ++-+U 1=0 12P =P 10Z = W=60j/kg f h 30/kg =2U =1m/s 2(60300.5)/g 3m Z =--=21Z Z Z 431m ∆=-=-=2. The fluid (density 1200 kg/m 3 ) is pumped at a constant rate 20 m 3 /h from the large reservoir to the evaporator. The pressure above the reservoir maintains atmosphere pressure and the pressure of the evaporator keeps 200 mmHg (vacuum). The distance between the level of liquid in the reservoir and the exit of evaporator is 15 meter and frictional loss in the pipe is 120 J/kg not including the exit of evaporator, what is the pump effective work and power if the diameter of pipe is 60 mm?22112212f U UZ g+W Z g+h 22ρρP P ++=++10P = 5422200P x1.013x10 2.67x10N /m 760=-=- 31200Kg /m ρ= 1U 0= f h 120J /kg =22V 20U 1.97m /s A 3600*4006===π/*. 1Z 0= 2Z 15=422.67x101.97W 15x9.81120246.88J /kg 12002=-+++=N W Q 246.88x1200x20/3600=1646W ρ==3. Water comes out of the pipe (Φ108x4 mm), as shown in Fig. The friction loss of the pipeline which does not cover the loss at the exit of pipe can be calculated by the following equation: h f =6.5U 2where U is the velocity in the pipe, find a. water velocity at section A-A'. b. water flow rate, in m 3 /h.22112212f U UZ g+Z g+h 22ρρP P +=++ 1U0= 12P =P 1Z 6m = 2Z 0=2f h 6.5U = 22U 6x9.81 6.5U 2=+U 2.9m/s = 23V=UA=2.94x01x360082m /h =π/.4. Water passes through the variable pipe. The velocity in the small pipe is 2.5 m/s. The vertical glass tubes are inserted respectively at the section A and B to measure the pressure (see fig.) If the friction loss between two section is 15 J/kg, what is the water column difference between two glass tubes? By the way, draw the relative liquid column height of two tubes in the Fig.a ab b U A U A = 2b U 2.5*(33/47)1.23m /s ==22aa b b a b f U U Z g+Z g+h 22ρρP P +=++ a b Z =Z22abb a f U U h 22ρρP P -=-+221.23/2 2.5/21512.63=-+= a b P P R g ρ-=∆ 3312.63R=1.29x10m 9.8x10-∆=5. A centrifugal pump takes brine (density 1180 kg/m 3 , viscosity 1.2 cp) from the bottom of a supply tank and delivers it into another tank. The line between the tanks is 300 m of 25 mm diameter pipe (inner diameter). The flow rate is 2 m 3 /h. In this line, there are two gate valves, four elbows (90o ) and one return bend, what is the friction loss if the roughness of pipe is 0.025 mm?22f fst flocal U U h h h 4f k d 22l ∑=+=+∑31180kg /m ρ= 300m, d=0.025m l =3-3v 2m /h =1.2cp=1.2x10Pa.s μ= k=0.025mm k/d=0.025/25=0.001 c l r k =0.4 k =1 k =2x0.07=0.14 el re k 4x0.75 3 k 1.5-2.2===2u v /A 2/(3600x /4x0.025)1.13m /s π===4u d Re 2.78x10ρμ== f 0.063=2f 2h 4x0.0063x300/0.025x1.13/2+(0.4+1+2x0.07+4x0.7+1.5)x1.13/2 =197.86J/kg∑=6. The orifice meter (diameter of orifice 0.0001 m) is installed for measuring the flow rate. The indicating liquid of orifice is mercury if U shape pressure gauge reading is 0.6 meterand orifice coefficient can be taken as 0.61, what is the flow rate of water?o u c =20o 0V u s 0.61x /4x0.0001π==835.8x10m /s -=7. Water flows through a pipe with a diameter di 100 mm as shown in figure.a. when the valve is closed, R is 600 mm and h equals 1500 mm. While the valve opens partially, R=400 mm and h=1400 mm, f=0.00625 (Finning factor) and k c =0.5 (contraction coefficient), what is the flow rate of water, in m 3 /h?b. If the valve opens fully, what is the pressure of section 2-2', in N/m 2 ? The equivalentlength of the valve is 1.5 m and the Fanning factor f keeps the same?(ρH2O =1000kg/m 3,ρHg =13600kg/m 3)(1) the valve opens partially ,for selection 1-1’ and 2-2’ , we have22112212f 1-2u u gZ gZ h 22ρρP P ++=+++ 2212Hg H o 0 g(R h)39630N/m ρρP =P =-= 2212f1-2c u u u 0 Z =0 h 4f +k 2.13ud 22===lWe can get Z1 from the valve closed21Hg H O h=1.5m R=0.6m Z gR/h 6.66m ρρ=-=229.81x6.66u /2 2.13u 39630/1000=++23h u=3.13m/s V 3600x /4x0.1x3.1388.5m /h π==(2) when the valve opens fully, for section 1-1’ and 3-3’, we have22331113f1-3u u gZ gZ h 22ρρP P ++=+++ 311Z 0 Z 6.66m u =0==22e f1-3c u 3.1.5h (4f k )(4x0.00625x +0.5) 4.81u d 20.01l l ++=+== 229.81x6.66u /2 4.81u =+ u 3.51m/s =For section 1-1’ and 2-2’22112212f1-2u u gZ gZ h 22ρρP P ++=+++112120 Z 6.66 Z 0 u 0 u 3.51P =====22f1-2c l u h (4f k )(4x0.00625x15/0.10.5)3.51/226.2J /kg d 2=+=+= 22229.81x6.66 3.15/226.2N32970mρP=++P =8. The rotameter is installed to measure the water flow rate, as shown in figure. If the total length including equivalent length of pipeline A is 10 m and the reading of rotameter is 2.72 m 3 /h, what is the flow rate for pipeline B? (f A =0.0075, f B =0.0045)For parallel pipe linefA fB total A B22A fAA 2A h h V V +V u (l+le) 2.72h 4f 4x0.0075x10/0.053/2()d 23600x /4x0.053π∑=∑=∑∑== 0.333J /kg =22B fB B B B 23B B B B u (l+le)h 4f 4x0.0045x2/0.3/2xu 0.333d 2u 2.36m /s V =u A 2.36x /4x0.23600m /hπ∑∑======10. A flat furnace wall is constructed of 120 mm layer of sil-o-cel brick, with a thermal conductivity 0.08 w/(m o C), backed by a 150 mm of common brick, of conductivity 0.8 w/(m o C), the temperature of inner face of the wall is 1400 o , and that of the outer face is 200o C. a. What is the heat loss through the wall in w per square meter.b. To reduce the heat loss to 600 w/m 2 by adding a layer of cork with k 0.2 w/(m o C) on the outside of common brick, how many meters of cork are requied?a. 2Q t 1400200711N /m 11L R 0.080.80.120.15∑∆-===∑+b. 600=(1400-200)/(0.12/0.08+0.15/0.8+x/0.2) x=0.0625m13. Air at the normal pressure passes through the pipe (d i 20 mm) and is heated from 20o C to 100o C. What is the film heat transfer coefficient between the air and pipe wall if the average velocity of air is 10 m/s? The properties of air at 60 o C are as follows:density 1.06 kg/m 3 , viscosity 0.02 cp, conductivity 0.0289 w/(m o C), and heat capacity 1kJ/kg-K443u d 10x0.02x1.06 Re=1.06x10100.02x10ρμ-==> 12T +T 20100T=6022+==℃ 0.141ωμμ⎛⎫= ⎪⎝⎭10000.020.0010.6920.0289p c x x k μ==Pr=()()0.81/3081/34Nu 0027Re Pr 0.027x 1.06x10x 0.69239.66==.=.()2i i i h d 39.66 h 39.66x0.0289/0.02=57.22w/m .k k==14. A hot fluid with a mass flow rate 2250 kg/h passes through a ∅25x2.5 mm tube. The physical properties of fluid are as follows:k=0.5 w/(m o C), C p =4 kJ/kg-K, viscosity 10-3 N-s/m 2 , density 1000 kg/m 3 Find: a. Heat transfer film coefficient h i , in w/(m 2 -K).b. If the flow rate decreases to 1125 kg/h and other conditions are the same, what is the h i ?c. If the diameter of tube (inside diameter) decreases to 10 mm, and the velocity u keeps the same as that of case a, calculate h i .d. When the average temperature of fluid and quantity of heat flow per meter of tube are 40 o C and 400 w/m, respectively, what is the average temperature of pipe wall for case a?e. From this problem, in order to increase the heat transfer film coefficient and enhance heat transfer, what kinds of methods can you use and which is better, explain? Hint: for laminar flow, Nu=1.86[Re Pr]1/3 for turbulent flow Nu=0.023Re 0.8 Pr 1/3(1) 444N 2250x4u d Gd d 3600x x0.02Re 3.98x10100.001ρππμμμ=====>()()1/30.8081/3424Nu 0023Re Pr 0.023x 3.98x10220.10.5Nuk 220.1x0.5hi 5500w /m k d 0.02⎛⎫== ⎪⎝⎭===.=.(2) 12w 2w = 4421Re Re /2=2x1010=>0.80.82211Nu Re 0.5Nu Re ⎛⎫== ⎪⎝⎭0.8i2i1h 0.5h = ()0.82i2h 5500x0.53159w /m k ==(3) 44333u d 2000x0.01Re 2x10100.001ρμ===>0.81/3Nu 0.023RePr = ()2hi=6347w/m k(4)i i w w Q=h A (t-t )=400=500x2x0.02(t-t )πw t=40t 39.41=℃ ℃(5) there methods : increase u or hi or decrease d The first is better15. In a double pipe exchange (Φ23x2 mm), the cold fluid (Cp=1 kJ/kg, flow rate 500 kg/h) passes through the pipe and the hot fluid goes through the outside. The inlet and outlet temperatures of cold fluid are 20 and 80 o , and the inlet and outlet temperatures of hot fluid are 150 and 90o , respectively. The h i (film coefficient inside pipe) is 700 w/(m 2 o C)and overall heat transfer coefficient U o (based on the outside surface of pipe) is 300w/(m 2 o C), respectively. If the heat loss is ignored and the conductivity of pipe wall (steel) is taken as 45 w/(m o C), find:(1) heat transfer film coefficient outside the pipe h o ? (2) the pipe length required for counter flow, in m?(3) what is the pipe length required if the heating medium changes to saturated vapor(140 o C) and it condenses to saturated liquid and other conditions keep unchanged? (4) When the exchanger is used for a year, it is found that it cannot meet the need of production (the outlet temperature of cold fluid cannot reach 80 o C), explain why?(a) 0m o 0i i m d l d 111230.002x23h Vo h d kd 300700x1945x21⎛⎫=-+=-- ⎪⎝⎭ 1/h0=1/U0-(do/hidi+bdo/kdm)=1/300-23/700*19-0.002*23/45*21 ()20h 642.9w/m k = 12t +t LMTD=702∆∆℃=Q=UoAo ∆Tm=mcCp(Tcb-Tca) 300*2π*0.023*70L=500/3600*1000*(80-20)L=5.4m(c) 8020LMTD=86.514020ln14080-=--℃1122L t70/86.5L t ∆==∆ 2L 0.81L1 4.4m ==(d) scale is formed on the outside ,V 0 is decreased16. Water flows turbulently in the pipe of Φ25x2.5 mm shell tube exchanger. When the velocity of water u is 1 m/s, overall heat transfer coefficient Uo (based on the outer surface areaof pipe) is 2115 w/(m 2o C). If the u becomes 1.5 m/s and other conditions keep unchanged, Uois 2660 w/( m 2o C ). What is the film coefficient ho outside the pipe? (Heat resistances of pipe wall and scale are ignored) o i h h Uo 111+= (1) oi o h h U 1'1'1+= (2) (1)-(2)=0.80.80.80.81211111121152660u C u C 1C 1.5C-=-=-C=2859io h Uo h 111-= ho=8127W/(m2K)17. Water and oil pass parallelly through an exchanger which is 1 m long. The inlet and outlet temperatures of water are 15 and 40 o C, and those of oil are 150 and 100 o C, respectively. If the outlet temperature of oil decreases to 80 o C, and the flow rates and physical properties and inlet temperatures of water and oil maintain the same, what is the pipe length of new exchanger? (Heat loss and pipe wall resistance are neglected)()()h h 12c c 21m Q W C T -T W C t t VA t ==-=∆()()h h 12c c 21W C T -T 'W C t 't =-2150100401515080t 15--=-- 2t 50=℃212m1112m2L T T 't 1508092.51.85L T T t 15010069.8-∆-===-∆- 2m1m2L 1.85m L1=1m t 92.5 t 69.8=∆=∆=18. Air which passes through the pipe in turbulent flow is heated from 20 to 80 o C. The saturatedvapor at 116.3 o C condenses to saturated water outside the pipe. If air flow rate increases to 120% of the origin and inlet and outlet temperatures of air stay constant, what kind of method can you employ in order to do that? (Heat resistance of pipe wall and scale can be ignored))(111ca cb pc c m i i T T C m T A h -=∆=1Q)'(2212ca cb pc c m i T T C m T A h -=∆=2Q 128.012112i22.12.1h m m c c m i m T T m m T h T ∆∆===∆∆ )803.116/()203.116ln(20801---=∆m T)80/()20ln(20802---=∆h h m T T TTh=118.5oC19. Water flows through the pipe of a Φ25x2.5 mm shell-tube exchanger from 20 to 50 o C. The hot fluid (C p 1.9 kJ/kg o C, flow rate 1.25 kg/s) goes along the shell and the temperatureschange from 80 to 30 o C. Film coefficients of water and hot fluid are 0.85kw/(m 2o C) and 1.7kw/(m 2o C). What is the overall heat transfer coefficient Uo and heat transfer area if the scale resistance can be ignored? (the conductivity of steel is 45w/(m o C).W=1.25Kg/s Cp=1.9Kj/kg ℃()()2h p 12Q W C T T 1.25x1.9x 80-30119Kw =-==m 3010t 30ln10-∆= ()200m 00i i m 1V 472w/m k d l d 1h h d kd ++==32i 0m Q 119x10A 13.9m V t 472x18.2===∆20. A spherical particle (density 2650 kg/m 3) settles freely in air at 20 o C (density of air 1.205 kg/m 3 , viscosity 1x10-5 Pa.s). Calculate the maximum diameter of particle if the settle obeys the Stoke s’ Law?Re ≤1 ()2p t p D g U 18D ρρμμρP -==()23p 18D g μρρρP =- ()1/3-10p 18x10D 1.205x9.81x 2650-1.205⎛⎫= ⎪ ⎪⎝⎭=3.85x10-521. A filter press(A=0.1 m 2 ) is used for filtering slurry. The vacuum inside the filter is 500 mm Hg. One liter filtrate can be got after filtering of 5 min and 0.6 more liter filtrate is obtained after 5 more min. How much filtrate will be got after filtering of 5 more min?for filter press 22e V 2VV =KA θ+5 min 22e 12V 0.1x5K +=(1) 10min 22e 1.62x1.6V 0.1x10K +=(2)From (1) (2),we can see Ve=0.7 K=4815 min 22V 2x0.7V=48x0.1x15+ V=2.07m 3/h22. The following data are obtained for a filter press (A=0.0093 m 2) in a lab.------------------------------------------------------------------------------------------------pressure difference (kg f /cm 2 ) filtering time (s) filtrate volume (m 3 )1.05 502.27⨯10-3 660 9.10⨯10-33.50 17.1 2.27⨯10-3 233 9.10⨯10-3 Find1) filtering constant K, q e , t e at pressure difference 1.05 kg f /cm 2 ?2) if the frame of filter is filled with the cake at 660 s, what is the end filtering rate (dV/dt)E at P 1.05 kg f /cm 2 ?3) compressible constant of cake s?For p=1.05Kg/cm 22e 2e 2e q 2qq K 0.002270.0002272x q 50K 0.00930.000930.000910.000912x q 660K 0.000930.00093θ+=⎛⎫+= ⎪⎝⎭⎛⎫+= ⎪⎝⎭We can see K=0.015 qe=0.026For p=3.5Kg/cm21-s K=2k ∆P 1-s K'=2k '∆P1sK 'K '-∆P ⎛⎫= ⎪∆P ⎝⎭ ()2E eV KA 2V+V d d θ⎛⎫=⎪⎝⎭23. A slurry is filtered by a 0.1 m 2 filter press at constant pressure if the cake is incompressible. The filter basic equation is as follows: (q+10)2 = 250(t+ 0.4) where q---l/m 2 t----minfind (1) how much filtrate is got after 249.6 min?(2) if the pressure difference is double and the resistance of cake is constant, how much filtrate can be obtained after 249.6 min? (cake is imcompressible)(1)let θ=249.6 ()()2q+10250x 249.60.4=+ q=240 V=qA=240*0.1=24 (2) K 2k =∆P K'2k '=∆P '2∆P =∆P K'2K 500== ()()2q'+10500x 249.60.4=+ q ’=343.6 v=34.36。
流体力学英文习题

图1-14 1-1 Water is moving through a pipe. The velocityprofile at some section is shown and is givenmathematically as)4(422r D V -=μβ where β= a constantr = radial distance from centerlineV = velocity at any position r Figure 1-11-2 What is the shear stress at the wall of the pipe from the water? What is the shear stress at a position 4/D r =? If the profile above persists a distance L along the pipe , What drag is induced on the pipe by the water in the direction of flow over this distance?Figure 1-21-3 cylinder of weight 90N slides in a lubricated pipe . The clearance between cylinder and pipe is 0.0245mm . If the cylinder is observed to decelerated at a rate of 0.61m/s 2 when the speed is 6.1m/s , what is the viscosity of the oil? The diameter of the cylinder D is 6.00 in(15.228cm) and the length L is 5.00 in(12.19cm).Figure 1-32-1 In Fig .2-1.A cylindrical tank contains water at aheight of 50mm . Inside is a smaller open cylindrical tankcontaining kerosene at height h having a specific gravityof 0.8.The following pressures are known from theindicated gages :B p =13.80 kPa gageC p =13.82 kPa gageWhat are the gage pressure A p and the height h of thekerosene ? Assume that the kerosene is prevented frommoving to the top of the tank . Figure 2-12-2 Determine the force and its position from fluids acting on the door in Fig .2-2.Figure 2-2 2-3 A cylindrical control weir is shown in Fig .2-65 .It has a diameter of 3m and a length of 6m . Give the magnitude and direction of the resultant force acting on the weir from the fluids .Figure 2-33-1 If friction is neglected, what is the velocity of the water issuing from the tank as a free jet? What is the discharge rate ?Figure 3-13-2 The way of measuring flow rate is to use the flow nozzle , which is a device inserted into the pipe as shown in Fig .3-2. If 2A is the exit area of the flow nozzle , show that for incompressible flow we get for q⎥⎥⎦⎤⎢⎢⎣⎡--=g p p g A A A C q d ρ2121222)/(1 where d C is the coefficient of discharge , which takes into account frictional effects and isdetermined experimentally .Figure 3-24-1 A flow is defined by x u 2= and y v 2-=.Find the stream function and potential function for this flow.4-2 Given the stream function y x 23-=ψ. Is this a potential flow? Does it satisfy the Laplace equation?5-1 What at 20℃ moves over one side of a flat plate . The free-stream speed is 3 m/s . The plate is 0.5 m wide . What maximum length should the plate be to have only a laminar boundary layer if the transition takes plate at x Re =5×105? For this length, what is the plate drag coefficient f C and the drag D F ?5-2 A body travels through air at 15℃ at a speed of 30 m/s , and 6kW is required to accomplish this . If the projected area is 3 m 2 in the direction of motion , determine the coefficient of drag . 6-1 As shown in Fig .6-1.What pressure 1p is needed to cause 100 L/s of water to flow into the device at a pressure 2p of 40 kPa gage? The pipe is 150 mm commercial pipe . Take ν=0.113×10-5m 2/s .Figure 6-16-2 Water is flowing through a pipe at the rate of 0.005m 3/s as shown in Fig.6-50.If the following gage pressures are measured, 1p =12kPa ,2p =11.5kPa ,3p =10.3kPa.What arethe head losses between 1 and 2 and between 1 and 3?Figure 6-27-1 An airplane is capable of attaining a flight Mach number of 0.8. When it is flying at an altitude of 1000 ft (304.88 m) in standard atmosphere, what is the ground speed if the air is not moving relative to the ground? What is the ground speed if the plane is at an altitude of 35000 ft (10670.73m) in standard atmosphere?7-2 Do the first part of Prob.7-11 if the air is moving at 60 km/h directly opposite to the direction of flight.7-3 Suppose that a plane is moving horizontally relative to the ground at a speed of twice the velocity of sound and that the air is moving in the opposite direction at a speed of one-half the velocity of sound relative to the ground. What is the Mach angle?。
流体力学第二版课后习题集规范标准答案

31.10体积为0.5 m的油料,重量为& m G g 4410 9.807 解:0.5 4410N,试求该油料的密度是多少? 899.358 (kg/m 3)第一章习题答案选择题(单选题)1.1按连续介质的概念,流体质点是指:(d )(a)流体的分子;(b)流体内的固体颗粒;(c)几何的点;(d)几何尺寸同流动空间相比是极小量,又含有大量分子的微元体。
1.2作用于流体的质量力包括:(c)(a)压力;(b)摩擦阻力;(c)重力;(d )表面张力。
1.3单位质量力的国际单位是:(d )2(a)N ;(b)Pa;(c)N / kg ;(d)m/s。
1.4与牛顿内摩擦定律直接有关的因素是:(b)(a)剪应力和压强;(b)剪应力和剪应变率;(c)剪应力和剪应变;(d )剪应力和流速。
1.5水的动力黏度□随温度的升高:(b )(a)增大;(b)减小;(c)不变;(d )不定。
1.6流体运动黏度的国际单位是:(a)2 O o(a)m/s ;(b)N /m ;(c)kg/m ;(d)N s/m。
1.7无黏性流体的特征是:(c)(a)黏度是常数;(b)不可压缩;(c )无黏性;(d )符合卫RT。
1.8 当水的压强增加1个大气压时,水的密度增大约为:(a)(a)1/20000 ;(b)1/10000 ;(c)1/4000 ;(d )1/2000。
31.9水的密度为1000 kg/m , 2L水的质量和重量是多少?解:m V 1000 0.002 2 (kg)G mg 2 9.807 19.614 (N)答:2L水的质量是2kg,重量是19.614N31.11 某液体的动力黏度为0.005 Pa s,其密度为850 kg / m,试求其运动黏度。
& 0.005 6 2解: 5.882 10 (m2/s)850答:其运动黏度为5.882 10 6m 2/s。
1.12 有一底面积为60cm X40cm的平板,质量为5Kg,沿一与水平面成20。
流体力学与传热习题参考解答(英文)

1. Water is pumped at a constant velocity 1m/s from large reservoir resting on the floor to the open top of an absorption tower. The point of discharge is 4 meter above the floor, and the friction losses from the reservoir to the tower amount to 30 J/kg. At what height in the reservoir must the water level be kept if the pump can develop only60 J/kg?2222112f 1U P U P w=Z g+h (Z g+)22ρρ++-+ U 1=0 12P =P 10Z = W=60j/kg f h 30/kg =2U =1m/s 2(60300.5)/g 3m Z =--=21Z Z Z 431m ∆=-=-=2. The fluid (density 1200 kg/m 3 ) is pumped at a constant rate 20 m 3 /h from the large reservoir to the evaporator. The pressure above the reservoir maintains atmosphere pressure and the pressure of the evaporator keeps 200 mmHg (vacuum). The distance between the level of liquid in the reservoir and the exit of evaporator is 15 meter and frictional loss in the pipe is 120 J/kg not including the exit of evaporator, what is the pump effective work and power if the diameter of pipe is 60 mm?22112212f U U Z g+W Z g+h 22ρρP P ++=++ 10P = 5422200P x1.013x10 2.67x10N /m 760=-=- 31200Kg /m ρ= 1U 0= f h 120J /kg =22V 20U 1.97m /s A 3600*4006===π/*. 1Z 0= 2Z 15= 422.67x101.97W 15x9.81120246.88J /kg 12002=-+++= N W Q 246.88x1200x20/3600=1646W ρ==3. Water comes out of the pipe (Φ108x4 mm), as shown in Fig. The friction loss of the pipeline which does not cover the loss at the exit of pipe can be calculated by the following equation:h f =6.5U 2where U is the velocity in the pipe, finda. water velocity at section A-A'.b. water flow rate, in m 3 /h. 22112212f U U Z g+Z g+h 22ρρP P +=++ 1U 0= 12P =P 1Z 6m = 2Z 0=2f h 6.5U = 22U 6x9.81 6.5U 2=+ U 2.9m/s = 23V=UA=2.94x01x360082m /h =π/.4. Water passes through the variable pipe. The velocity in the small pipe is 2.5 m/s. The vertical glass tubes are inserted respectively at the section A and B to measure the pressure (see fig.) If the friction loss between two section is 15 J/kg, what is the water column difference between two glass tubes? By the way, draw the relative liquid column height of two tubes in the Fig.a ab b U A U A = 2b U 2.5*(33/47)1.23m /s == 22a a b b a b f U U Z g+Z g+h 22ρρP P +=++ a b Z =Z 22a b b a f U U h 22ρρP P -=-+221.23/2 2.5/21512.63=-+= a b P P R g ρ-=∆ 3312.63R=1.29x10m 9.8x10-∆=5. A centrifugal pump takes brine (density 1180 kg/m 3 , viscosity 1.2 cp) from the bottom of a supply tankand delivers it into another tank. The line between the tanks is 300 m of 25 mm diameter pipe (inner diameter). The flow rate is 2 m 3 /h. In this line, there are two gate valves, four elbows (90o ) and one return bend, what is the friction loss if the roughness of pipe is 0.025 mm?22f fst flocal U U h h h 4f k d 22l ∑=+=+∑ 31180kg /m ρ= 300m, d=0.025m l =3-3v 2m /h =1.2cp=1.2x10Pa.s μ=k=0.025mm k/d=0.025/25=0.001c l r k =0.4 k =1 k =2x0.07=0.14el re k 4x0.75 3 k 1.5-2.2===2u v /A 2/(3600x /4x0.025)1.13m /s π===4u d Re 2.78x10ρμ== f 0.063= 2f 2h 4x0.0063x300/0.025x1.13/2+(0.4+1+2x0.07+4x0.7+1.5)x1.13/2 =197.86J/kg∑=6. The orifice meter (diameter of orifice 0.0001 m) is installed for measuring the flow rate. The indicating liquid of orifice is mercury if U shape pressure gauge reading is 0.6 meter and orifice coefficient can be takenas 0.61, what is the flow rate of water?o u c =20o 0V u s 0.61x /4x0.0001π==835.8x10m /s -=7. Water flows through a pipe with a diameter di 100 mm as shown in figure.a. when the valve is closed, R is 600 mm and h equals 1500 mm. While the valve opens partially, R=400 mm and h=1400 mm, f=0.00625 (Finning factor) and k c =0.5 (contraction coefficient), what is the flow rate of water, in m 3 /h?b. If the valve opens fully, what is the pressure of section 2-2', in N/m 2 ? The equivalent length of the valve is1.5 m and the Fanning factor f keeps the same?(ρH2O =1000kg/m 3, ρHg =13600kg/m 3)(1) the valve opens partially ,for selection 1-1’ and 2-2’ , we have 22112212f 1-2u u gZ gZ h 22ρρP P ++=+++ 2212Hg H o 0 g(R h)39630N/m ρρP =P =-= 2212f1-2c u u u 0 Z =0 h 4f +k 2.13u d 22===l We can get Z1 from the valve closed21Hg H O h=1.5m R=0.6m Z gR/h 6.66m ρρ=-=229.81x6.66u /2 2.13u 39630/1000=++23h u=3.13m/s V 3600x /4x0.1x3.1388.5m /h π==(2) when the valve opens fully, for section 1-1’ and 3-3’, we have 22331113f1-3u u gZ gZ h 22ρρP P ++=+++ 311Z 0 Z 6.66m u =0== 22e f1-3c u 3.1.5h (4f k )(4x0.00625x +0.5) 4.81u d 20.01l l ++=+== 229.81x6.66u /2 4.81u =+ u 3.51m/s =For section 1-1’ and 2-2’22112212f1-2u u gZ gZ h 22ρρP P ++=+++ 112120 Z 6.66 Z 0 u 0 u 3.51P ===== 22f1-2c l u h (4f k )(4x0.00625x15/0.10.5)3.51/226.2J /kg d 2=+=+= 22229.81x6.66 3.15/226.2N 32970mρP =++P =8. The rotameter is installed to measure the water flow rate, as shown in figure. If the total length including equivalent length of pipeline A is 10 m and the reading of rotameter is 2.72 m 3 /h, what is the flow rate for pipeline B? (f A =0.0075, f B =0.0045)For parallel pipe line fA fB total A B22A fA A 2A h h V V +V u (l+le) 2.72h 4f 4x0.0075x10/0.053/2()d 23600x /4x0.053π∑=∑=∑∑== 0.333J /kg = 22B fB B B B 23B B B B u (l+le)h 4f 4x0.0045x2/0.3/2xu 0.333d 2u 2.36m /s V =u A 2.36x /4x0.23600m /h π∑∑======10. A flat furnace wall is constructed of 120 mm layer of sil-o-cel brick, with a thermal conductivity 0.08 w/(m o C), backed by a 150 mm of common brick, of conductivity 0.8 w/(m o C), the temperature of inner face of the wall is 1400 o , and that of the outer face is 200o C.a. What is the heat loss through the wall in w per square meter.b. To reduce the heat loss to 600 w/m 2 by adding a layer of cork with k 0.2 w/(m o C) on the outside of common brick, how many meters of cork are requied? a. 2Q t 1400200711N /m 11L R 0.080.80.120.15∑∆-===∑+ b. 600=(1400-200)/(0.12/0.08+0.15/0.8+x/0.2)x=0.0625m13. Air at the normal pressure passes through the pipe (d i 20 mm) and is heated from 20o C to 100o C. What is the film heat transfer coefficient between the air and pipe wall if the average velocity of air is 10 m/s? The properties of air at 60 o C are as follows:density 1.06 kg/m 3 , viscosity 0.02 cp, conductivity 0.0289 w/(m o C), and heat capacity 1 kJ/kg-K443u d 10x0.02x1.06 Re=1.06x10100.02x10ρμ-==>12T +T 20100T=6022+==℃ 0.141ωμμ⎛⎫= ⎪⎝⎭10000.020.0010.6920.0289p c x x k μ==Pr= ()()0.81/3081/34Nu 0027Re Pr 0.027x 1.06x10x 0.69239.66==.=. ()2i i i h d 39.66 h 39.66x0.0289/0.02=57.22w/m .k k ==14. A hot fluid with a mass flow rate 2250 kg/h passes through a ∅25x2.5 mm tube. The physical properties of fluid are as follows:k=0.5 w/(m o C), C p =4 kJ/kg-K, viscosity 10-3 N-s/m 2 , density 1000 kg/m 3 Find:a. Heat transfer film coefficient h i , in w/(m 2 -K).b. If the flow rate decreases to 1125 kg/h and other conditions are the same, what is the h i ?c. If the diameter of tube (inside diameter) decreases to 10 mm, and the velocity u keeps the same as that of case a, calculate h i .d. When the average temperature of fluid and quantity of heat flow per meter of tube are 40 o C and 400 w/m, respectively, what is the average temperature of pipe wall for case a?e. From this problem, in order to increase the heat transfer film coefficient and enhance heat transfer, what kinds of methods can you use and which is better, explain?Hint: for laminar flow, Nu=1.86[Re Pr]1/3for turbulent flow Nu=0.023Re 0.8 Pr 1/3 (1) 444N 2250x4u d Gd d 3600x x0.02Re 3.98x10100.001ρππμμμ=====> ()()1/30.8081/3424Nu 0023Re Pr 0.023x 3.98x10220.10.5Nuk 220.1x0.5hi 5500w /m k d 0.02⎛⎫== ⎪⎝⎭===.=. (2) 12w 2w = 4421Re Re /2=2x1010=> 0.80.82211Nu Re 0.5Nu Re ⎛⎫== ⎪⎝⎭ 0.8i2i1h 0.5h = ()0.82i2h 5500x0.53159w /m k == (3) 44333u d 2000x0.01Re 2x10100.001ρμ===> 0.81/3Nu 0.023Re Pr = ()2hi=6347w/m k(4)i i w w Q=h A (t-t )=400=500x2x0.02(t-t )πw t=40t 39.41=℃ ℃(5) there methods : increase u or hi or decrease dThe first is better15. In a double pipe exchange (Φ23x2 mm), the cold fluid (Cp=1 kJ/kg, flow rate 500 kg/h) passes throughthe pipe and the hot fluid goes through the outside. The inlet and outlet temperatures of cold fluid are 20 and 80 o , and the inlet and outlet temperatures of hot fluid are 150 and 90o , respectively. The h i (film coefficient inside pipe) is 700 w/(m 2 o C)and overall heat transfer coefficient U o (based on the outside surface of pipe) is 300w/(m 2 o C), respectively. If the heat loss is ignored and the conductivity of pipe wall (steel) is taken as 45 w/(m o C), find:(1) heat transfer film coefficient outside the pipe h o ?(2) the pipe length required for counter flow, in m?(3) what is the pipe length required if the heating medium changes to saturated vapor(140 o C) and it condenses to saturated liquid and other conditions keep unchanged?(4) When the exchanger is used for a year, it is found that it cannot meet the need of production (the outlet temperature of cold fluid cannot reach 80 o C), explain why? (a) 0m o 0i i m d l d 111230.002x23h Vo h d kd 300700x1945x21⎛⎫=-+=-- ⎪⎝⎭ 1/h0=1/U0-(do/hidi+bdo/kdm)=1/300-23/700*19-0.002*23/45*21()20h 642.9w/m k =12t +t LMTD=702∆∆℃= Q=UoAo ∆Tm=mcCp(Tcb-Tca) 300*2π*0.023*70L=500/3600*1000*(80-20)L=5.4m(c) 8020LMTD=86.514020ln 14080-=--℃ 1122L t 70/86.5L t ∆==∆ 2L 0.81L1 4.4m == (d) scale is formed on the outside ,V 0 is decreased16. Water flows turbulently in the pipe of Φ25x2.5 mm shell tube exchanger. When the velocity of water u is 1 m/s, overall heat transfer coefficient Uo (based on the outer surface area of pipe) is 2115 w/(m 2 o C). If the u becomes 1.5 m/s and other conditions keep unchanged, Uo is 2660 w/( m 2 o C ). What is the film coefficient ho outside the pipe? (Heat resistances of pipe wall and scale are ignored)o i h h Uo 111+= (1) oi o h h U 1'1'1+= (2) (1)-(2)= 0.80.80.80.81211111121152660u C u C 1C 1.5C-=-=- C=2859 io h Uo h 111-= ho=8127W/(m2K)17. Water and oil pass parallelly through an exchanger which is 1 m long. The inlet and outlet temperatures of water are 15 and 40 o C, and those of oil are 150 and 100 o C, respectively. If the outlet temperature of oil decreases to 80 o C, and the flow rates and physical properties and inlet temperatures of water and oil maintain the same, what is the pipe length of new exchanger? (Heat loss and pipe wall resistance are neglected) ()()h h 12c c 21m Q W C T -T W C t t VA t ==-=∆()()h h 12c c 21W C T -T 'W C t 't =-2150100401515080t 15--=-- 2t 50=℃ 212m1112m2L T T 't 1508092.51.85L T T t 15010069.8-∆-===-∆- 2m1m2L 1.85m L1=1m t 92.5 t 69.8=∆=∆=18. Air which passes through the pipe in turbulent flow is heated from 20 to 80 o C. The saturated vapor at 116.3 o C condenses to saturated water outside the pipe. If air flow rate increases to 120% of the origin and inlet and outlet temperatures of air stay constant, what kind of method can you employ in order to do that? (Heat resistance of pipe wall and scale can be ignored))(111ca cb pc c m i i T T C m T A h -=∆=1Q)'(2212ca cb pc c m i T T C m T A h -=∆=2Q 128.012112i22.12.1h m m c c m i m T T m m T h T ∆∆===∆∆ )803.116/()203.116ln(20801---=∆m T )80/()20ln(20802---=∆h h m T T T Th=118.5oC19. Water flows through the pipe of a Φ25x2.5 mm shell-tube exchanger from 20 to 50 o C. The hot fluid (C p1.9 kJ/kg o C, flow rate 1.25 kg/s) goes along the shell and the temperatures change from 80 to 30 o C. Film coefficients of water and hot fluid are 0.85kw/(m 2 o C) and 1.7 kw/(m 2 o C). What is the overall heat transfer coefficient Uo and heat transfer area if the scale resistance can be ignored? (the conductivity of steel is 45w/(m o C).W=1.25Kg/s Cp=1.9Kj/kg ℃()()2h p 12Q W C T T 1.25x1.9x 80-30119Kw =-==m 3010t 30ln 10-∆= ()200m 00i i m 1V 472w/m k d l d 1h h d kd ++==32i 0m Q 119x10A 13.9m V t 472x18.2===∆20. A spherical particle (density 2650 kg/m 3) settles freely in air at 20 o C (density of air 1.205 kg/m 3 , viscosity 1x10-5 Pa.s). Calculate the maximum diameter of particle if the settle obeys the Stoke s’ Law?Re ≤1 ()2p t p D g U 18D ρρμμρP -== ()23p 18D g μρρρP =- ()1/3-10p 18x10D 1.205x9.81x 2650-1.205⎛⎫= ⎪ ⎪⎝⎭=3.85x10-521. A filter press(A=0.1 m 2 ) is used for filtering slurry. The vacuum inside the filter is 500 mm Hg. One liter filtrate can be got after filtering of 5 min and 0.6 more liter filtrate is obtained after 5 more min. How much filtrate will be got after filtering of 5 more min?for filter press 22e V 2VV =KA θ+5 min 22e 12V 0.1x5K +=(1)10min 22e 1.62x1.6V 0.1x10K +=(2)From (1) (2),we can see Ve=0.7 K=4815 min 22V 2x0.7V=48x0.1x15+ V=2.07m 3/h22. The following data are obtained for a filter press (A=0.0093 m 2) in a lab.------------------------------------------------------------------------------------------------pressure difference (kg f /cm 2 ) filtering time (s) filtrate volume (m 3 )1.05 502.27⨯10-3660 9.10⨯10-33.50 17.1 2.27⨯10-3233 9.10⨯10-3Find1) filtering constant K, q e , t e at pressure difference 1.05 kg f /cm 2 ?2) if the frame of filter is filled with the cake at 660 s, what is the end filtering rate (dV/dt)E at P 1.05 kg f /cm 2 ?3) compressible constant of cake s?For p=1.05Kg/cm 22e 2e 2e q 2qq K 0.002270.0002272x q 50K 0.00930.000930.000910.000912x q 660K 0.000930.00093θ+=⎛⎫+= ⎪⎝⎭⎛⎫+= ⎪⎝⎭We can see K=0.015 qe=0.026For p=3.5Kg/cm 21-s K=2k ∆P 1-s K'=2k '∆P 1s K 'K '-∆P ⎛⎫= ⎪∆P ⎝⎭ ()2E e V KA 2V+V d d θ⎛⎫= ⎪⎝⎭23. A slurry is filtered by a 0.1 m 2 filter press at constant pressure if the cake is incompressible. The filter basic equation is as follows:(q+10)2 = 250(t+ 0.4)where q---l/m 2 t----minfind (1) how much filtrate is got after 249.6 min?(2) if the pressure difference is double and the resistance of cake is constant, how much filtrate can be obtained after 249.6 min? (cake is imcompressible)(1)let θ=249.6 ()()2q+10250x 249.60.4=+ q=240 V=qA=240*0.1=24(2) K 2k =∆P K'2k '=∆P'2∆P =∆P K'2K 500== ()()2q'+10500x 249.60.4=+ q ’=343.6 v=34.36。
流体力学课后习题习题答案

2-1 2.94fwd ρρ== 2-2222222222230.135 1.976 2.9270.003 1.4290.052 1.2510.760.8040.051.341/CO CO SO SO O O N N H O H Okg m ρραραραραρα=++++=⨯+⨯+⨯+⨯+⨯= 2-3302732730.8109/10132527310132527317010085814321.341p kg m t ρρ=⨯=++-=⨯223250273()10273 1.57CO CO S T Pa s T S μμ-+=+=⨯223250273()10273 1.854SO SO S T Pa s T S μμ-+=+=⨯223250273()10273 2.781O O S T Pa s T S μμ-+=+=⨯22350273()102732.365N N S T Pa s T S μμ-+=+=⨯223250273()10273 1.62H O H O S T Pa s T S μμ-+=+=⨯1212121212222222222222221212121212222222222252.2310CO CO CO SO SO SO O O O N N N H O H O H O CO CO SO SO O O N N H O H OM M M M M M M M M M Pa sαμαμαμαμαμμααααα-++++=++++=⨯ 5210/2.75m s μνρ-==⨯ 2-4 8500002.5100.0002dp dp Pa dV V d ρρK =-===⨯ 2-5(1)52 3.92310(/)dp dp dpP Pa RT dV V d RT p V dp p VK =-=-=-=-=⨯(2)等熵过程有pv C γ=1511 5.492101(/)/dp dp dp Pa dV V d C p V p dp Vp γγγγ--K =-=-===⨯ 2-6 mV ρ∆∆=3153//p V m V V p V∆K K =-⇒=-=∆∆∆2-13 轴承和轴之间间隙很小,可近似认为速度在此处呈直线分布,由牛顿内摩擦定律,有()2250.760du r F Adl dy D d n P kW P Fv F r ωμμππωω⎫==⎪-⎪⎪=⇒=⎬⎪==⎪⎪⎭2-14 本题中飞轮受到惯性力和轴承中摩擦力的作用,对飞轮列出定轴转动微分方程,有 d J M dtω'=- (J 为飞轮矩,M '为摩擦力矩,G 为飞轮重力,r 为轴半径) 2604n GD d duA rg dt dyrdlrπωμμπδ=-=-代入相应数值后得0.2328Pa s μ=5-2 (1)30.1520l l h h h m h k k ''=⨯===⇒ (2)这是重力作用下的不可压流动了,此时满足Fr 数相等。
最新流体力学第三章课后习题答案

一元流体动力学基础1•直径为150mm的给水管道,输水量为980・7kN/h,试求断面平均流速。
(kN/h —> kg/s => Q = QV A)注意:Qv=—-pA得:v= 1.57m/s2.斷面为SOOnimX^Omm的矩形风道,风量为2700m,/h,求平均流速.如风道出口处斷面收缩为150mmX 400mm,求该断面的平均流速Q 解:由流量公式Q = VA得:AV=由连续性方程知V1A =V2A2得:v2 =12.5111/s3.水从水箱流经直径dxhOcn, 25cm, ds=2. 5cm的管道流入大气中.当出口流速10m/时,求(1)容积流量及质量流量;(2)山及d?管段的流速解.(1)由Q == 0.0049m3 /s质量流量^=4-9kg/s(2)由连续性方程:V] A = A,\‘2 A = \‘3 A得:v i = 0・625m/ s,v2 = 2.5m/ s4•设计输水量为294210kg h的给水管道,流速限制在0.9sl.4m/s之间。
试确定管道直径,根据所选直径求流速.直径应是56口口的倍数.解:Q = pvA 将v= 0.9 co 14m/s 代入得d = 0.343 s 0.275m・・•直径是50111111的倍数,所以取d = 0.3m代入Q = "A 得v= 1.18m5.圆形风道,流量是10000m7h,,流速不超过20m/s・试设计直径,根据所定直径求流速. 直径规定为50 mm的倍数。
解:Q = \'A 将v V 20in/ s 代入得:d > 420.5niin 取 d = 450nm】代入Q = vA 得:17.5m/s7•某蒸汽管干管的始端蒸汽流速为25 m/s,密度为2. 62 kg/『.干管前段宜径为50皿, 接出宜径40皿支管后,干管后段直径改为45 nm.如果支管末端密度降为2・30 kg/m 、, 干管后段末端密度降为2・24 kg/n 3,但两管质量流量相同,求两管终端流速.Qi :=Q 支解,由題意可得2 Jv 皿=18m/ s 得:£支=22.2m/s8. 空气流速由超音速过渡到亚超音速时,要经过冲击波。
流体力学第1、2、3、4章课后习题答案

GAGGAGAGGAFFFFAFAF第一章習題答案選擇題(單選題)1.1按連續介質的概念,流體質點是指:(d )(a )流體的分子;(b )流體內的固體顆粒;(c )幾何的點;(d )幾何尺寸同流動空間相比是極小量,又含有大量分子的微元體。
1.2作用于流體的質量力包括:(c )(a )壓力;(b )摩擦阻力;(c )重力;(d )表面張力。
1.3單位質量力的國際單位是:(d )(a )N ;(b )Pa ;(c )kg N /;(d )2/s m 。
1.4與牛頓內摩擦定律直接有關的因素是:(b )(a )剪應力和壓強;(b )剪應力和剪應變率;(c )剪應力和剪應變;(d )剪應力和流速。
1.5水的動力黏度μ隨溫度的升高:(b )(a )增大;(b )減小;(c )不變;(d )不定。
1.6流體運動黏度ν的國際單位是:(a )(a )2/s m ;(b )2/m N ;(c )m kg /;(d )2/m s N ⋅。
1.7無黏性流體的特征是:(c )(a )黏度是常數;(b )不可壓縮;(c )無黏性;(d )符合RT p =ρ。
GAGGAGAGGAFFFFAFAF1.8當水的壓強增加1個大氣壓時,水的密度增大約為:(a ) (a )1/20000;(b )1/10000;(c )1/4000;(d )1/2000。
1.9 水的密度為10003kg/m ,2L 水的質量和重量是多少?解: 10000.0022m V ρ==⨯=(kg )29.80719.614G mg ==⨯=(N )答:2L 水的質量是2 kg ,重量是19.614N 。
1.10 體積為0.53m 的油料,重量為4410N ,試求該油料的密度是多少? 解: 44109.807899.3580.5m Gg VVρ====(kg/m 3)答:該油料的密度是899.358 kg/m 3。
1.11某液體的動力黏度為0.005Pa s ⋅,其密度為8503/kg m ,試求其運動黏度。
《流体力学》课后习题答案详解
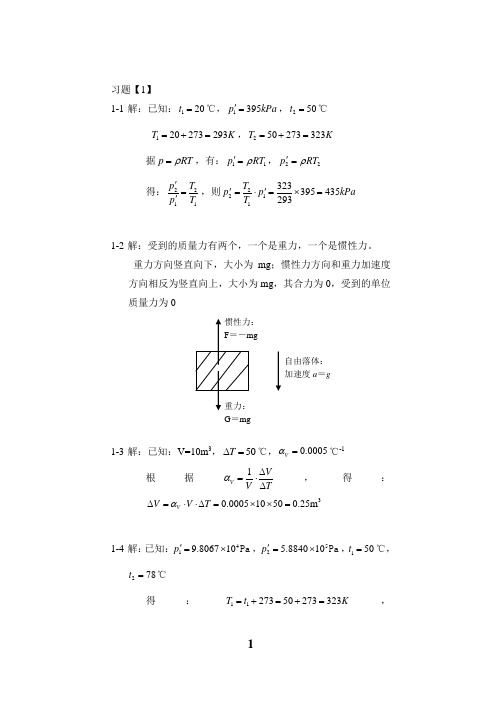
习题【1】1-1 解:已知:120t =℃,1395p kPa '=,250t =℃ 120273293T K =+=,250273323T K =+= 据p RT ρ=,有:11p RT ρ'=,22p RT ρ'= 得:2211p T p T '=',则2211323395435293T p p kPa T ''=⋅=⨯=1-2 解:受到的质量力有两个,一个是重力,一个是惯性力。
重力方向竖直向下,大小为mg ;惯性力方向和重力加速度方向相反为竖直向上,大小为mg ,其合力为0,受到的单位质量力为01-3 解:已知:V=10m 3,50T ∆=℃,0.0005V α=℃-1根据1V V V Tα∆=⋅∆,得:30.000510VVV Tα∆=⋅⋅∆=⨯⨯1-4 解:已知:419.806710Pa p '=⨯,52 5.884010Pa p '=⨯,150t =℃,278t =℃得:1127350273323T t K=+=+=,G =mg自由落体: 加速度a =g2227378273351T t K =+=+=根据mRTp V=,有:111mRT p V '=,222mRT p V '=得:421251219.8067103510.185.884010323V p T V p T '⨯=⋅=⨯='⨯,即210.18V V = 体积减小了()10.18100%82%-⨯=1-5 解:已知:40mm δ=,0.7Pa s μ=⋅,a =60mm ,u =15m/s ,h =10mm根据牛顿内摩擦力定律:uT Ayμ∆=∆ 设平板宽度为b ,则平板面积0.06A a b b =⋅=上表面单位宽度受到的内摩擦力:1100.70.06150210.040.01T A u b N b b h b μτδ-⨯-==⋅=⨯=--/m ,方向水平向左下表面单位宽度受到的内摩擦力: 2200.70.061506300.010T A u b N b b h b μτ-⨯-==⋅=⨯=--/m ,方向水平向左平板单位宽度上受到的阻力:12216384N τττ=+=+=,方向水平向左。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
1.1 What will be the (a) the gauge pressure and (b) the absolute pressure of water at depth 12mbelow the surface? ρwater = 1000 kg/m 3, and P atmosphere = 101kN/m 2.Solution:Rearranging the equation 1.1-4gh p p a b ρ+=Set the pressure of atmosphere to be zero, then the gauge pressure at depth 12m below the surfaceiskPa gh p p a b 72.1171281.910000=⨯⨯+=+=ρAbsolute pressure of water at depth 12mkPa Pa gh p p a b 72.2182187201281.91000101000==⨯⨯+=+=ρ1.3 A differential manometer as shown in Fig. is sometimes used to measure small pressuredifference. When the reading is zero, the levels in two reservoirs are equal. Assume that fluid B ismethane (甲烷), that liquid C in the reservoirs is kerosene (specific gravity = 0.815), and thatliquid A in the U tube is water. The inside diameters of the reservoirs and U tube are 51mm and6.5mm , respectively. If the reading of the manometer is145mm., what is the pressure differenceover the instrument In meters of water, (a) when the change in the level in the reservoirs isneglected, (b) when the change in the levels in the reservoirs is taken into account? What is thepercent error in the answer to the part (a)?Solution :p a =1000kg/m 3 p c =815kg/m 3 p b =0.77kg/m 3 D/d=8 R=0.145mWhen the pressure difference between two reservoirs is increased, the volumetric changes in the reservoirs and U tubesR d x D 2244ππ= (1) so R D d x 2⎪⎭⎫ ⎝⎛= (2) and hydrostatic equilibrium gives following relationshipg R g x p g R p A c c ρρρ++=+21 (3)sog R g x p p c A c )(21ρρρ-+=- (4)substituting the equation (2) for x into equation (4) givesg R g R D d p p c A c )(221ρρρ-+⎪⎭⎫ ⎝⎛=- (5) (a )when the change in the level in the reservoirs is neglected,()Pa g R g R g R D d p p c A c A c 26381.98151000145.0)()(221=⨯-=-≈-+⎪⎭⎫ ⎝⎛=-ρρρρρ(b )when the change in the levels in the reservoirs is taken into account()Pa g R g R D d g R g R D d p p c A c c A c 8.28181.98151000145.081.9815145.0515.6)()(22221=⨯-+⨯⨯⨯⎪⎭⎫ ⎝⎛=-+⎪⎭⎫ ⎝⎛=-+⎪⎭⎫ ⎝⎛=-ρρρρρρ error=%=7.68.2812638.281- 1.4 There are two U-tube manometers fixed on the fluid bed reactor, as shown in the figure. The readings of two U-tube manometers are R 1=400mm ,R 2=50mm, respectively. The indicating liquid is mercury. The top of the manometer is filled with the water to prevent from the mercury vapor diffusing into the air, and the height R 3=50mm. Try to calculate the pressure at point A and B .Solution: There is a gaseous mixture in the U-tube manometer meter. The densities of fluids are denoted by Hg O H g ρρρ,,2, respectively. The pressure at point A is given by hydrostatic equilibriumFigure for problem 1.4g R R g R g R p g Hg O H A )(32232+-+=ρρρg ρis small and negligible in comparison with Hg ρand ρH2O , equation above can be simplifiedc A p p ≈=232gR gR Hg O H ρρ+=1000×9.81×0.05+13600×9.81×0.05=7161N/m²1gR p p p Hg A D B ρ+=≈=7161+13600×9.81×0.4=60527N/m1.5 Water discharges from the reservoir through the drainpipe, which the throat diameter is d. The ratio of D to d equals 1.25. The vertical distance h between the tank A and axis of the drainpipe is 2m. What height H from the centerline of the drainpipe to the water level in reservoir is required for drawing the water from the tank A to the throat of the pipe? Assume that fluid flow is a potential flow. The reservoir, tank A and the exit ofdrainpipe are all open to air.Solution: Bernoulli equation is written between stations 1-1 and 2-2, with station 2-2 being reference plane: 2222222111u gz p u gz p ++=++ρρ Where p 1=0, p 2=0, and u 1=0, simplification of the equation22u Hg =1The relationship between the velocity at outlet and velocity u o at throat can be derived by the continuity equation:22⎪⎭⎫ ⎝⎛=⎪⎪⎭⎫ ⎝⎛D d u u o 22⎪⎭⎫ ⎝⎛=d D u u o 2 Bernoulli equation is written between the throat and the station 2-23 Combining equation 1,2,and 3 givesSolving for HH=1.39m1.6 A liquid with a constant density ρ kg/m 3 is flowing at an unknown velocity V 1 m/s through a horizontal pipe of cross-sectional area A 1 m 2 at a pressure p 1 N/m 2, and then it passes to a section of the pipe in which the area is reduced gradually to A 2 m 2 and the pressure is p2. Assuming no friction losses, calculate the velocities V 1 and V 2 if the pressure difference (p 1 - p 2) is measured. Solution :In Fig1.6, the flow diagram is shown with pressure taps to measure p 1 and p 2. From the mass-balance continuity equation , for constant ρ where ρ1 = ρ2 = ρ,222200u u p =+ρ()===144.281.92100081.910002125.11112442-⨯⨯⨯--⎪⎭⎫ ⎝⎛==ρρg h d D u Hg2112A A V V = For the items in the Bernoulli equation , for a horizontal pipe,z 1=z 2=0Then Bernoulli equation becomes, after substituting 2112A A V V = for V 2, ρρ22121211212020p A A V p V ++=++ Rearranging,2)1(21212121-=-A A V p p ρ ⎥⎥⎦⎤⎢⎢⎣⎡-⎪⎪⎭⎫ ⎝⎛-12221211A A p p V ρ=Performing the same derivation but in terms of V 2,⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛--21221212A A p p V ρ=1.7 A liquid whose coefficient of viscosity is µ flows below the critical velocity for laminar flow in a circular pipe of diameter d and with mean velocity V . Show that the pressure loss in a length of pipe L p ∆ is 232d V μ. Oil of viscosity 0.05 Pas flows through a pipe of diameter 0.1m with a average velocity of 0.6m/s.Calculate the loss of pressure in a length of 120m.Solution :The average velocity V for a cross section is found by summing up all the velocities over the cross section and dividing by the cross-sectional area1From velocity profile equation for laminar flow2 substituting equation 2 for u into equation 1 and integrating3rearranging equation 3 gives1.8. In a vertical pipe carrying water, pressure gauges areinserted at points A and B where the pipe diameters are0.15m and 0.075m respectively. The point B is 2.5m belowA and when the flow rate down the pipe is 0.02 m 3/s, thepressure at B is 14715 N/m 2 greater than that at A.Assuming the losses in the pipe between A and B can be expressed as g V k 22where V is the velocity at A, find the value of k .If the gauges at A and B are replaced by tubes filled with water and connected to a U-tube containing mercury of relative density 13.6, give a sketch showing how the levels in the two limbs of the U-tube differ and calculate the value of this difference in metres.Solution:d A =0.15m; d B =0.075mz A -z B =l =2.5mQ =0.02 m 3/s,p B -p A =14715 N/m 2 Figure for problem 1.8 ⎰⎰==R R rdr u R udA A V 020211ππ⎪⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛--=22014R r R L p p u L μ2032D L p p V L μ-=232d V L p μ=∆Pa d VL p 115201.01206.005.0323222=⨯⨯⨯==∆μs m d QV V d Q AA AA /132.115.0785.002.044222=⨯===ππsm d Q V V d Q BB BB /529.4075.0785.002.044222=⨯===ππWhen the fluid flows down, writing mechanical balance equation222222AB B B A A A V k V g z p Vg z p +++=++ρρ213.1253.4100014715213.181.95.2222k ++=+⨯k 638.0260.10715.14638.0525.24++=+=k 0.295making the static equilibriumgR g x g l p g R g x p Hg A B ρρρρρ+∆++=+∆+()()mm g g l p p R g H A B 7981.91260081.910005.214715-=⨯⨯⨯-=---=ρρρ1.9.The liquid vertically flows down through the tube from thestation a to the station b , then horizontally through the tube fromthe station c to the station d , as shown in figure. Two segments ofthe tube, both ab and cd ,have the same length, the diameter androughness.Find:(1)the expressions of g p ab ρ∆, h fab , g pcdρ∆and h fcd , respectively.(2)the relationship between readings R 1and R 2 in the U tube.Solution:(1) From Fanning equationFigure for problem 1.92V l h fab λ=andsoFluid flows from station a to station b , mechanical energy conservation giveshence2from station c to station dhence3From static equationp a -p b =R 1(ρˊ-ρ)g -l ρg 4p c -p d =R 2(ρˊ-ρ)g5 Substituting equation 4 in equation 2 ,thentherefore6Substituting equation 5 in equation 3 ,then7ThusR 1=R 222V d l h fcd λ=fcdfab h h =fab b a h p p+=+ρρlg fab b a h p p =+-lg ρfcddc h pp +=ρρfcd d c h p p =-ρfabh g l g R =+--'lg 1ρρρρ)(g R h fab ρρρ-'=1g R h fcd ρρρ-'=21.10 Water passes through a pipe of diameter d i=0.004 m with the average velocity 0.4 m/s, as shown in Figure.1) What is the pressure drop –∆P when water flows through the pipe length L =2 m, in m H 2O column?2) Find the maximum velocity and point r at which it occurs.3) Find the point r at which the average velocityequals the local velocity. 4)if kerosene flows through this pipe ,how do thevariables above change ?(the viscosity and density of Water are 0.001 Pasand 1000 kg/m 3,respectively ;and the viscosityand density of kerosene are 0.003 Pas and 800kg/m 3,respectively )solution:1)1600001.01000004.04.0Re =⨯⨯==μρud from Hagen-Poiseuille equation1600004.0001.024.0323222=⨯⨯⨯==∆d uL P μ m g p h 163.081.910001600=⨯=∆=ρ 2)maximum velocity occurs at the center of pipe, from equation 1.4-19max 0.5V u = so u max =0.4×2=0.8m3)when u=V=0.4m/s Eq. 1.4-172max 1⎪⎪⎭⎫ ⎝⎛-=wr r u u 5.0004.01max2=⎪⎭⎫ ⎝⎛-u V r = m r 00284.071.0004.05.0004.0=⨯== 4) kerosene:427003.0800004.04.0Re =⨯⨯==μρud Pa pp 4800001.0003.01600=='∆='∆μμFigure for problem 1.10m g p h 611.081.98004800=⨯=''∆='ρ1.12 As shown in the figure, the water level in the reservoir keeps constant. A steel drainpipe (with the inside diameter of 100mm) is connected to the bottom of the reservoir. One arm of the U-tube manometer is connected to the drainpipe at the position 15m away from the bottom of the reservoir, and the other is opened to the air, the U tube is filled with mercury and the left-side arm of the U tube above the mercury is filled with water. The distance between the upstream tap and the outlet of the pipeline is 20m.a) When the gate valve is closed, R=600mm, h=1500mm; when the gate valve is opened partly, R=400mm, h=1400mm. The friction coefficient λ is 0.025, and the lo ss coefficient of the entrance is 0.5. Calculate the flow rate of water when the gate valve is opened partly. (in m³/h)b) When the gate valve is widely open, calculate the static pressure at the tap (in gauge pressure, N/m²). l e /d ≈15 when the gate valve is widely open, and the friction coefficient λ is still 0.025.Solution :(1) When the gate valve is opened partially, the water discharge isSet up Bernoulli equation between the surface of reservoir 1—1’ and the section of pressure point 2—2’,and take the center of section 2—2’ as the referring plane, then∑+++=++21,2222121122—f h p u gZ p u gZ ρρ (a ) In the equation 01=p (the gauge pressure)222/396304.181.910004.081.913600m N gh gR p O H Hg =⨯⨯-⨯⨯=-=ρρ Figure for problem 1.120021=≈Z uWhen the gate valve is fully closed, the height of water level in the reservoir can be related to h (the distance between the center of pipe and the meniscus of left arm of U tube).gR h Z g Hg O H ρρ=+)(12 (b )where h=1.5mR=0.6mSubstitute the known variables into equation b 2222_1,113.22)5.01.015025.0(2)(66.65.110006.013600V V V K d l h m Z c f =+⨯=+==-⨯=∑λ Substitute the known variables equation a9.81×6.66=2213.21000396302V V ++ the velocity is V =3.13m/sthe flow rate of water is h m V d V h /5.8813.312.0436004360032=⨯⨯⨯=⨯=ππ2) the pressure of the point where pressure is measured when the gate valve is wide-open. Write mechanical energy balance equation between the stations 1—1’ and 3-3´,then∑+++=++31,3233121122—f h p V gZ p V gZ ρρ (c ) since m Z 66.61=311300p p u Z =≈=2223_1,81.4 2]5.0)151.035(025.0[ 2)(V V V K d l l h c e f =++=++=∑λ input the above data into equation c ,9.8122V 81.4266.6+=⨯Vthe velocity is: V =3.51 m/sWrite mechanical energy balance equation between thestations 1—1’ and 2——2’, for the same situation of water level ∑+++=++21,2222121122—f h p V gZ p V gZ ρρ (d )since m Z 66.61=212103.51/0(page pressure Z u u m s p =≈≈=)kg J V K d l hc f /2.26251.3)5.01.015025.0(2)(222_1,=+⨯=+=∑λ input the above data into equationd , 9.81×6.66=2.261000251.322++p the pressure is: 329702=p1.14 Water at 20℃ passes through a steel pipe with an inside diameter of 300mm and 2m long. There is a attached-pipe (Φ60⨯3.5mm) which is parallel with the main pipe. The total length including the equivalent length of all form losses of the attached-pipe is 10m. A rotameter is installed in the branch pipe. When the reading of the rotameter is2.72m 3/h, try to calculate the flow rate in the main pipe and the total flow rate, respectively. The frictional coefficient of the main pipe and the attached-pipe is 0.018 and 0.03, respectively.Solution : The variables of main pipe are denoted by a subscript1, and branch pipe by subscript 2.The friction loss for parallel pipelines is2121S S s f f V V V h h +==∑∑The energy loss in the branch pipe is 22222222u d l l h e f ∑∑+=λ In the equation 03.02=λs m u d ml l e /343.0053.04360072.2053.01022222=⨯⨯===+∑πinput the data into equation ckg J h f /333.02343.0053.01003.022=⨯⨯=∑The energy loss in the main pipe is 333.022111121===∑∑u d l h h f f λ So s m u /36.22018.023.0333.01=⨯⨯⨯= The water discharge of main pipe ish m V h /60136.23.043600321=⨯⨯⨯=π Total water discharge ish m V h /7.60372.26013≈+=1.16 A Venturimeter is used for measuring flow of water along a pipe. The diameter of the Venturi throat is two fifths the diameter of the pipe. The inlet and throat are connected by water filled tubes to a mercury U-tube manometer. The velocity of flow along the pipe is found to beR 5.2 m/s, where R is the manometer reading in metres of mercury. Determine the loss of head between inlet and throat of the Venturi when R is 0.49m. (Relative density of mercury is 13.6). Solution:Writing mechanical energy balance equation between the inlet1 and throat o for Venturi meterf o o hg z V p g z V p +++=++22121122ρρ 1 rearranging the equation above, and set (z 2-z 1)=xf o oh xg V V p p ++-=-22121ρ 2 from continuity equationFigure for problem 1.1611221125.625V V d d V V o o =⎪⎭⎫ ⎝⎛=⎪⎪⎭⎫ ⎝⎛= 3 substituting equation 3 for V o into equation 2 gives()f f f f oh xg R h xg R h V h xg V V p p ++=++=+=++-=-94.1185.203.1903.19206.3922121211ρ 4from the hydrostatic equilibrium for manometerg x g R p p Hg o ρρρ+-=-)(1 5 substituting equation 5 for pressure difference into equation 4 obtainsf Hgh xg R gx g R ++=+-94.118)(ρρρρ 6 rearranging equation 6 kg J R R R R g R h Hg f /288.267.494.11861.12394.118)(==-=--=ρρρ1.17.Sulphuric acid of specific gravity 1.3 is flowing through a pipe of 50 mm internal diameter. A thin-lipped orifice, 10mm, is fitted in the pipe and the differential pressure shown by a mercury manometer is 10cm. Assuming that the leads to the manometer are filled with the acid,calculate (a)the weight of acid flowing per second, and (b) the approximate friction loss in pressure caused by the orifice.The coefficient of the orifice may be taken as 0.61, the specific gravity of mercury as 13.6, and the density of water as 1000 kg/m 3Solution: a)2.0501010==D D =⨯-=-=-81.9)130013600(1.0)(21g R p p Hg ρρ()s m p p D D C V o /63.231.461.056.1861.0130081.9)130013600(1.022.0161.021*******=⨯=≈⨯-⨯-=-⎪⎪⎭⎫ ⎝⎛-=ρs kg V D m /268.0130063.201.0442220=⨯⨯⨯==πρπb) approximate pressure drop=⨯-=-=-81.9)130013600(1.0)(21g R p p Hg ρρ12066.3Pa pressure difference due to increase of velocity in passing through the orificePa D D V V V V p p o 8.44882)2.01(63.213002242412222212221=-=⎪⎪⎭⎫ ⎝⎛-=-=-ρρ pressure drop caused by friction lossPa p f 5.75778.44883.12066=-=∆2.1 Water is used to test for the performances of pump. The gauge pressure at the discharge connection is 152 kPa and the reading of vacuum gauge at the suction connection of the pump is 24.7 kPa as the flow rate is 26m 3/h. The shaft power is 2.45kw while the centrifugal pump operates at the speed of 2900r/min. If the vertical distance between the suction connection and discharge connection is 0.4m, the diameters of both the suction and discharge line are the same. Calculate the mechanical efficiency of pump and list the performance of the pump under this operating condition.Solution:Write the mechanical energy balance equation between the suction connection and discharge connection 2_1,2222121122f H gp g u Z H g p g u Z +++=+++ρρ wherem Z Z 4.012=-(Pa 1052.1(Pa 1047.22_1,215241≈=⨯=⨯-=f H u u pressure gauge p pressure gauge p ))total heads of pump is m H 41.1881.9100010247.01052.14.055=⨯⨯+⨯+= efficiency of pump is N N e /=ηsince kW g QH N e 3.1360081.9100041.18263600=⨯⨯⨯==ρN=2.45kWThen mechanical efficiency %1.53%10045.23.1=⨯=η The performance of pump is Flow rate ,m³/h26 Total heads ,m18.41 Shaft power ,kW2.45 Efficiency ,%53.12.2 Water is transported by apump from reactor, which has200 mm Hg vacuum, to thetank, in which the gaugepressure is 0.5 kgf/cm 2, asshown in Fig. The totalequivalent length of pipe is200 m including all localfrictional loss. The pipeline isφ57×3.5 mm , the orificecoefficient of C o and orificediameter d o are 0.62 and 25mm, respectively. Frictional coefficient λ is 0.025. Calculate: Developed head H of pump, in m (the reading R of U pressure gauge in orifice meter is 168 mm Hg)Solution:Equation(1.6-9)Mass flow rates kg S V m o o /02.21000025.0414.312.42=⨯⨯⨯==ρ 2) Fluid flow through the pipe from the reactor to tank, the Bernoulli equation is as follows for V 1=V 2f H z gp p H +∆+-=ρ12 ∆z=10ms m Rg D d C V f /12.444.69375.062.01000)100013600(81.9168.025025162.02144000=⨯=-⨯⨯⎪⎭⎫ ⎝⎛-=-⎪⎭⎫ ⎝⎛-=ρρρ)(Pa p 7570710013.17602001081.95.054=⨯⨯+⨯⨯=∆ ∆p/ρg=7.7mThe relation between the hole velocity and velocity of pipeFriction losssoH=7.7+10+5.1=22.8m2.3 . A centrifugal pump is to be used to extract water from a condenser in which the vacuum is 640 mm of mercury, as shown in figure. At the rated discharge, the netpositive suction head must be at least 3m above the cavitation vaporpressure of 710mm mercury vacuum. If losses in the suction pipeaccounted for a head of 1.5m. What must be the least height of the liquid level in the condenser above the pump inlet?Solution :From an energy balance,WhereP o =760-640=120mmHgP v =760-710=50mmHgUse of the equation will give the minimum height H g as2.4 Sulphuric acid is pumped at 3 kg/s through a 60m length of smooth 25 mm pipe. Calculate the drop in pressure. If the pressure drop falls by one half, what will the new flowrate be ?• Density of acid 1840kg/m 3• Viscosity of acid 25×10-3 PasSolution:Velocity of acid in the pipe:s m D d V V /12112.42200=⎪⎭⎫ ⎝⎛⨯=⎪⎭⎫ ⎝⎛=m g u d l f H f 1.581.92105.0200025.02422=⨯⨯==NPSH H g p p H f v og ---=ρm NPSH H g p p H f v o g 55.335.181.9100081.913600)05.012.0-=--⨯⨯⨯-=---=(ρs m d m d mpipe of area tional cross flowrate volumetric u /32.3025.01840785.03785.04sec 222=⨯⨯===-=ρπρReynolds number:6109102532.31840025.0Re 3=⨯⨯⨯==-μρud from Fig.1.22 for a smooth pipe when Re=6109, f=0.0085 pressure drop is calculated from equation 1.4-9kg J u d l f ph f /450232.3025.0600085.042422=⨯==∆=ρ kPa p 5.8271840450=⨯=∆ or friction factor is calculated from equation1.4-25kg J u d l u d l f ph f /426232.3025.0606109046.042Re 046.042422.022.02=⨯⨯⨯==∆=--=ρkPa p 84.7831840426=⨯=∆ if the pressure drop falls to 783.84/2=391.92kPa8.18.12.12.038.12.12.022.0012.089.1079`2025.060102518401840046.042046.042Re 046.043919202u u u d l u d l p p =⎪⎭⎫ ⎝⎛⨯⨯⨯=⎪⎪⎭⎫ ⎝⎛⨯⨯⨯==∆='∆----ρμρρ= so s m u /27.236.489..1079012.03919208.18.1==⨯= new mass flowrate=0.785d 2u ρ=0.785×0.0252×2.27×1840=2.05kg/s2.4 Sulphuric acid is pumped at 3 kg/s through a 60m length of smooth 25 mm pipe. Calculate the drop in pressure. If the pressure drop falls by one half on assumption that the change of friction factor is negligible, what will the new flowrate be ?Density of acid 1840kg/m 3Viscosity of acid 25×10-3 Pa Friction factor 32.0Re 500.00056.0+=f for hydraulically smooth pipe Solution:Write energy balance equation:f h gu z g p H g u z g p +++=+++2222222111ρρ gu d l g p h H f 22λρ=∆== 342=ρπu ds m d u /32.31840025.014.3124322=⨯⨯=⨯=ρπ 611510251840025.032.3Re 3=⨯⨯⨯=- 0087.061155.00056.0Re 500.00056.032.032.0=+=+=f 92.4681.9232.3025.0600087.04222=⨯⨯==∆==g u d l g p h H f λρ Δp=46.92×1840×9.81=847.0kpa2.6 The fluid is pumped through the horizontal pipe from section A to B with the φ38⨯2.5mm diameter and length of 30 meters, shown as figure. The orifice meter of 16.4mm diameter is used to measure the flow rate. Orifice coefficient C o =0.63. the permanent loss in pressure is3.5×104N/m 2, the friction coefficient λ=0.024. find:(1) What is the pressure drop along the pipe AB?(2)What is the ratio of power obliterated in pipe AB to total power supplied to the fluid when the shaft work is 500W, 60%efficiency? (The density of fluid is 870kg/m 3 )solution :∑+++=+++f A A A A AA h u p g z w u p g z 2222ρρ ρλρ022p u d l h p p f BA ∆+==-∑247.0334.162=⎪⎭⎫ ⎝⎛=A A o()()s m gR C u /5.8870870136006.081.9297.063.02247.01200=-⨯⨯=''--=ρρρ ∴u = (16.4/33)2×8.5=2.1m/s∴242/76855105.321.2033.030870024.0m N h p p f B A =⨯+⨯==-∑ρ(2)W u d p Wm 1381.2033.0785.0768554Ne 22=⨯⨯⨯=∆==ρπρsothe ratio of power obliterated in friction losses in AB to total power supplied to the fluid%%=461006.0500138⨯⨯。
