Asymptotic expansion of holonomic distributions of one complex variable

合集下载

Holomorphic functions of slow growth on nested covering spaces of compact manifolds

a r X i v :m a t h /9808042v 2 [m a t h .C V ] 3 M a r 1999HOLOMORPHIC FUNCTIONS OF SLOW GROWTHON NESTED COVERING SPACES OF COMPACT MANIFOLDS Finnur L ´a russon University of Western Ontario 3March 1999Abstract.Let Y be an inﬁnite covering space of a projective manifold M in P N of di-mension n ≥2.Let C be the intersection with M of at most n −1generic hypersurfaces of degree d in P N .The preimage X of C in Y is a connected submanifold.Let φbe the smoothed distance from a ﬁxed point in Y in a metric pulled up from M .Let O φ(X )be the Hilbert space of holomorphic functions f on X such that f 2e −φis integrable on X ,and deﬁne O φ(Y )similarly.Our main result is that (under more general hypotheses than described here)the restriction O φ(Y )→O φ(X )is an isomorphism for d large enough.This yields new examples of Riemann surfaces and domains of holomorphy in C n with corona.We consider the important special case when Y is the unit ball B in C n ,and show that for d large enough,every bounded holomorphic function on X extends to a unique function in the intersection of all the nontrivial weighted Bergman spaces on B .Finally,assuming that the covering group is arithmetic,we establish three dichotomies concerning the extension of bounded holomorphic and harmonic functions from X to B .Introduction Let Y →M be an inﬁnite covering space of an n -dimensional projective manifold,n ≥2.The function theory of such spaces is still not well understood.The central problem in this area is the conjecture of Shafarevich that the universal covering space of anyprojective manifold is holomorphically convex.This is a higher-dimensional variation on the venerable theme of uniformization.There are no known counterexamples to the conjecture,and it has been veriﬁed only in a number of fairly special cases.Suppose M is embedded into a projective space by sections of a very ample line bundle L .The generic linear subspace of codimension n −1intersects M in a 1-dimensionalconnected submanifold C called an L-curve.The preimage X of C is a connected Riemann surface embedded in Y.A natural approach to constructing holomorphic functions on Y is to extend them from X.This has the advantage of reducing certain questions to the1-dimensional case,but the price one pays is having to work with functions of slow growth.Here,slow growth means slow exponential growth with respect to the distance from aﬁxed base point or a similar well-behaved exhaustion,in an L2or L∞sense. Functions in the Hardy class H p(X)grow slowly in this sense for p large enough.In Section1,we improve upon the main result of our earlier paper[L´a r1]and show that if L is suﬃciently ample,then the restriction map·|X is an isomorphism of the Hilbert spaces of holomorphic functions of slow growth.As before,the proof is based on the L2 method of solving the¯∂-equation.This may be viewed as a sampling and interpolation theorem,related to those of Seip;Berndtsson and Ortega Cerd`a;and others.See[BO], [Sei],and the references therein.In Section2,we use the isomorphism theorem to construct new examples of Riemann surfaces with corona.These easily deﬁned surfaces have many symmetries,we have a simple description of characters in the corona,and the corona is large in the sense that it contains a domain in euclidean space of arbitrarily high dimension.In Section3,we adapt results of H¨o rmander on generating algebras of holomorphic functions of exponential growth to the case of covering spaces.Consequently,as shown in Section4,the restriction map·|X may fail to be an isomorphism if L is not suﬃciently ample compared to the exhaustion.Under the mild assumption that the covering group is Gromov hyperbolic,we found in[L´a r2]that the only obstruction to every positive harmonic function on X being the real part of a holomorphic function(in which case X has many holomorphic functions of slow growth that extend to Y)is a geometric condition involving the Martin boundary, characteristic of the higher-dimensional case.There are examples of inﬁnitely connected X for which the obstruction is not present,but these have1-dimensional boundary, whereas in general the curves X of interest to us do not:they have the same boundary as the ambient space Y.No examples with higher dimensional boundary are known.In hopes of shedding some light on the dichotomy in[L´a r2],we restrict ourselves from Section4onwards to what seems to be the most auspicious setting possible and let Y be the unit ball B in C n,n≥2.We present the results of the previous sections in a more explicit form.We obtain a sampling and interpolation theorem for the weighted Bergman spaces on B.For each weight,the restriction to X induces an isomorphism from the weighted Bergman space on B to the one on X if L is suﬃciently ample.This is in contrast to Seip’s result that no sequence in the disc is both sampling and interpolating for any weighted Bergman space[Sei].Also,every bounded holomorphic function on X extends to a unique function of just barely exponential growth on B,i.e.,a function in the intersection of all the nontrivial weighted Bergman spaces on B,when L is suﬃciently ample,for instance when L is the m-th tensor power of the canonical bundle K with m≥2.Whether the extension is itself bounded is an important open question.2In Section5,assuming that the covering group is an arithmetic subgroup of the au-tomorphism group P U(1,n)of B,we establish two dichotomies related to that in[L´a r2] but using very diﬀerent means.One of them says that either every holomorphic function f continuous up to the boundary on the preimage of a K⊗m-curve in aﬁnite covering of M extends to a continuous function onsoX |f|2e−φ≤cY|f|2e−φ.This shows that we have a continuous linear restriction mapρ:Oφ(Y)→Oφ(X),f→f|X.In a previous paper we showed that under suitable curvature assumptions,ρis surjec-tive when n≥2.1.1.Theorem[L´a r1,Thm.3.1].If n≥2andΘ≥i∂¯∂φ+εωfor someε>0,thenρis surjective.By[L´a r1,Cor.2.4],since the weighted metric eφh in L has curvature−i∂¯∂φ+Θ≥εω, the k-th L2cohomology group H k(2)(Y,L∨)of Y with coeﬃcients in the dual bundle L∨with the dual metric e−φh∨vanishes for k<n.The proof of Theorem1.1is based on vanishing for k=1,for which we need n≥2.We will now use vanishing for k=0to show thatρis injective.Let f∈Oφ(Y)such that f|X=0.Thenα=fs∨is a holomorphic section of L∨on Y.We will show thatαis square-integrable with respect to e−φh∨.Then vanishing ofH0(2)(Y,L∨)implies thatα=0,so f=0.Since∇s=0on C,there is a constant c>0such that dist(·,C)≤c|s|on M.For y∈Y\X let x∈X have dist(y,x)=dist(y,X).Then|α(y)|=|f(y)||s(y)|−1≤c|f(x)−f(y)|/dist(x,y)≤c sup|d f|≤cB|f|≤c B|f|2 1/2,where the supremum is taken over a ball centred at y covering all of M,and B is a ballof larger radius.Hence,|α(y)|2e−φ(y)≤cB|f|2e−φ,soY |α|2e−φ≤cY|f|2e−φ<∞.We have proved the following theorem.1.2.Theorem.SupposeΘ≥i∂¯∂φ+εωfor someε>0.Thenρis injective.If dim X≥1,thenρis an isomorphism.By induction,the theorem generalizes to the case when C is the common zero locus of sections s1,...,s k,k≤n,of L over M which,in a trivialization,can be completed to4a set of local coordinates at each point of C.When k=n−1,such C will be referred to as L-curves.If L is very ample,and therefore the pullback of the hyperplane bundle by an embedding of M into some projective space,then this condition means that the linear subspace{s1,...,s k=0}intersects M transversely in a smooth subvariety C of codimension k.By Bertini’s theorem,this holds for the generic linear subspace of codimension k.If k≤n−1,then C is connected and the mapπ1(C)→π1(M)is surjective by the Lefschetz hyperplane theorem,which implies that X is connected.An important example of a functionφas above is obtained by smoothing the distance δfrom aﬁxed point in Y.By a result of Napier[Nap],there is a smooth functionτon Y such that(1)c1δ≤τ≤c2δ+c3for some c1,c2,c3>0,(2)dτis bounded,and(3)i∂¯∂τis bounded.Furthermore,by(1)and since the curvature of Y is bounded below,there is c>0such that e−cτis integrable on Y.Then e−cτis also integrable on X.If L is positive,then kΘ≥i∂¯∂τfor k∈N suﬃciently large by(3),so the curvature inequality in Theorem1.2holds if L is replaced by a suﬃciently high tensor power of itself.1.3.Example.There is an open hyperbolic Riemann surface X such that for some ε>0,any f∈O(X)with|f|≤ceεδis constant,whereδis the distance from aﬁxed point in the Poincar´e metric.Namely,there is an example due to Cousin of a projective2-dimensional torus(abelian surface)M with a Z-covering space Y→M such that Y has no nonconstant holomorphic functions[NR,3.9].Letτbe a smooth function on Y satisfying(1),(2),and(3),such that e−τ/2is integrable.Let L be a very ample line bundle on M such thatΘ≥i∂¯∂τ+εω. Let X be the pullback in Y of an L-curve in M.Then Oτ(X)=C by Theorem1.2.If f∈O(X)and|f|≤ceεδwithε>0suﬃciently small,then|f|≤ceτ/4,so f∈Oτ(X) and f is constant.2.New examples of Riemann surfaces with coronaLet X be a complex manifold.Let H∞(X)be the space of bounded holomorphic functions on X,which is a Banach algebra in the supremum norm.Let M be the character space of H∞(X),which is a compact Hausdorﬀspace in the weak-star topology.There is a continuous mapι:X→M taking x∈X to the evaluation character f→f(x).The Corona Problem asks whetherι(X)is dense in M.The complement of the closure of ι(X)in M is referred to as the corona,so ifι(X)is not dense in M,then X is said to have corona.It is well known that the following are equivalent.(1)ι(X)is dense in M.(2)If f1,...,f m∈H∞(X)and|f1|+···+|f m|>ε>0,then there are g1,...,g m∈H∞(X)such that f1g1+···+f m g m=1.5By Carleson’s famous Corona Theorem(1962),the disc has no corona.The Corona The-orem holds for Riemann surfaces ofﬁnite type and planar domains of various kinds.The Corona Problem for arbitrary planar domains is open.Around1970,Cole constructed theﬁrst example of a Riemann surface with corona[Gam].By modifying Cole’s exam-ple,Nakai obtained a regular Parreau-Widom surface with corona[Nak],[Has,p.229]. Recently,Barrett and Diller showed that the homology covering spaces of domains in the Riemann sphere,whose complement has positive logarithmic capacity and zero length, have corona[BD].See also[EP,7.3].Sibony[Sib]found theﬁrst example of a domain of holomorphy in C n,n≥2,with corona.There are no known examples of such domains without corona.We will now present a new class of Riemann surfaces with corona(see also Theorem 4.2).We remind the reader that if Y is a bounded domain in C n covering a compact complex manifold M,then Y is a domain of holomorphy[Sie,p.136]and M is projective. In fact,the canonical bundle of M is ample[Kol,5.22].2.1.Theorem.Letπ:Y→M be a covering map,where Y is a bounded domain in C n, n≥2,and M is compact.Let L be an ample line bundle on M.If C is an L⊗m-curve in M with m suﬃciently large,then the Riemann surface X=π−1(C)has corona.In fact, the natural map from X into the character space M of H∞(X)extends to an embedding of Y into M which maps Y\X into the corona of X.Proof.Letτ≥0be a smoothed distance function on Y as described in Section1,such that e−τis integrable on Y,and hence on X,so H∞(X)⊂Oτ(X).We claim that Oτ(X)·Oτ(X)⊂O3τ(X).Namely,suppose f∈Oτ(X).For p∈X, let B be the ball of radius1centred at p in a metric pulled up from C.Then|f(p)|≤cB|f|≤c B|f|2 1/2,where the constants are independent of p,so|f(p)|e−τ(p)/2≤c B|f|2e−τ 1/2<∞.Hence,|f|≤ceτ/2,soX |f|4e−3τ≤cXe2τe−3τ<∞,and f2∈O3τ(X).If m is suﬃciently large,then the curvature of L⊗m is at least3i∂¯∂τ+ωfor some K¨a hler formωon M,so the restriction mapρ:O3τ(Y)→O3τ(X)is an isomorphism by Theorem 1.2.Clearly,ρOτ(Y)=Oτ(X).For p∈Y,letλp be the linear functional f→ρ−1(f)(p) on H∞(X).If f,g∈H∞(X),thenρ−1(f)ρ−1(g)∈Oτ(X)·Oτ(X)⊂O3τ(X),and6ρ−1(f)ρ−1(g)|X=fg,soρ−1(f)ρ−1(g)=ρ−1(fg).This shows thatλp is a character on H∞(X).We have obtained a mapι:Y→M,p→λp,extending the natural map from X into M.We claim thatιis a homeomorphism onto its image with the induced topology,and thatι(X)∩ι(Y)=ι(X).This shows that the corona of X contains an embedded image of Y\X.Let us remark that a Riemann surface as in the theorem is not Parreau-Widom,since a Parreau-Widom surface X embeds into the character space of H∞(X)as an open subset [Has,p.222].See also the proof of Theorem5.2.By the same argument we easily obtain the following more general result.2.2.Theorem.Let Y be a covering space of a projective manifold M with dim M≥2, L be a line bundle on M,and C be an L-curve in M with preimage X in Y.If(1)L is suﬃciently ample,and(2)there is a bounded holomorphic map g:Y→C m withU,then we can show by the same argument as in the proof of Theorem2.1that U has corona.Since X is Stein,U may be chosen to be a domain of holomorphy by Siu’s theorem[Siu].Thus we obtain new examples of bounded domains of holomorphy in C n with corona.3.Generating H¨o rmander algebras on covering spacesIn this section,we adapt results of H¨o rmander[H¨o r]on generating algebras of holo-morphic functions of exponential growth to the case of covering spaces over compact manifolds.We let X→M be a covering space of an n-dimensional compact hermitian manifold M.Letφ:X→[0,∞)be a smooth function such that(1)dφis bounded,and(2)e−cφis integrable on X for some c>0.LetAφ=Aφ(X)= c>0O cφ(X)7be the vector space of holomorphic functions f on X such that f2e−cφis integrable on X for some c>0.By(2),Aφcontains all bounded holomorphic functions on X.The following is easy to see by an argument similar to that in the proof of Theorem2.1.3.1.Proposition.A holomorphic function f on X is in Aφif and only if|f|≤ce aφfor some a>0.Hence,Aφis a C-algebra,called a H¨o rmander algebra.If functions f1,...,f m in Aφgenerate Aφ,then there are g1,...,g m∈Aφsuch that f1g1+···+f m g m=1,somaxi=1,...,m|f i|≥ce−aφfor some a>0.We will establish an eﬀective converse to this observation.Our proof is a straightforward adaptation of H¨o rmander’s Koszul complex argument in[H¨o r].See also[EP,7.3]. Let m≥1and r,s≥0be integers,and t∈[0,∞).Choose a basis{e1,...,e m} for C m.Let L s r(t)be the space of smoothΛs C m-valued(0,r)-forms on X which are square-integrable with respect to e−tφ.3.2.Lemma.Suppose M is K¨a hler with K¨a hler formω,and Ric(X)+ti∂¯∂φ≥εωfor someε>0.Ifη∈L s r+1(t)and¯∂η=0,then there isξ∈L s r(t)with¯∂ξ=η.Proof.This follows directly from standard L2theory.See for instance[Dem,Sec.14].Now let f1,...,f m be holomorphic functions on X such thatce−c2φ≤max i|f i|2≤ce c1φ,c1,c2>0.Deﬁne a linear operatorα:L s+1r(t)→L s r(t+c1)by the formulaα(e i1∧···∧e i s+1)=s+1k=1(−1)k+1f ike i1∧···∧ˆe i k∧···∧e i s+1,and setα=0on L0r(t).Thenα2=0,andαcommutes with¯∂.Also deﬁne a linear operatorβ:L s r(t)→L s+1r(t+c1+2c2)by the formulaβ(ξ)=13.3.Lemma.Suppose e−aφis integrable on X.Ifξ∈L s r(t)andα(ξ)=0,then thereisη∈L s+1r (t+c1+2c2)such thatα(η)=ξand in addition¯∂η∈L s+1r+1(t+2c1+3c2+a)if¯∂ξ=0.Proof.Takeη=β(ξ).Say¯∂ξ=0.Then|¯∂η|≤c|ξ|max i ¯∂¯f iThen functions f1,...,f m in Aφgenerate Aφif and only if−aφfor some a>0.maxi=1,...,m|f i|≥ceThe hypotheses of the corollary are satisﬁed for example when X is the unit ball in C n andφ=−log(1−|·|2),which is comparable to the Bergman distance from the origin (see Section4).Proof of Theorem3.4.If s≥m or r>n,thenξ=0and we takeη=0.Assume that s<m and r≤n,and that the theorem has been proved with r,s replaced by r+1,s+1.By Lemma3.3,there isη1∈L s+1r(t+c1+2c2)such thatα(η1)=ξand¯∂η1∈L s+1r+1(t+2c1+3c2+a).Now¯∂¯∂η1=0andα(¯∂η1)=¯∂α(η1)=¯∂ξ=0, so by the induction hypothesis,there isη2∈L s+2r+1(u−c1)such that¯∂η2=0and α(η2)=¯∂η1.By Lemma3.2,there isη3∈L s+2r(u−c1)such that¯∂η3=η2.Now letη=η1−α(η3)∈L s+1r(u).Then¯∂η=¯∂η1−α(¯∂η3)=¯∂η1−α(η2)=0and α(η)=α(η1)=ξ.4.The case of the ballIn this section,we will consider the results of the previous sections in the explicit setting of the unit ball B in C n,n≥2.For an instructive discussion of compact ball quotients,see[Kol,Ch.8].Let M be a projective manifold covered by B with a positive line bundle L with curvatureΘ.Let X be the preimage in B of an L-curve C in M.We are particularly interested in the extension problem for bounded holomorphic functions on X.The restriction map H∞(B)→H∞(X)is injective,so we can consider H∞(B)as a subspace of H∞(X),which is closed in the sup-norm.In the locally uniform topology, however,H∞(B)is dense in H∞(X).Namely,say f∈H∞(X),and let F∈O(B)be an extension of f.For r<1,the functions z→F(rz)are bounded in B,and they converge locally uniformly to f on X as r→1.It is well known that if Y is a complex submanifold of a neighbourhood ofwhereg jk= ∂∂z k =n+12Im ds2=i2∂¯∂log(1−|z|2).The distance from the origin in the Bergman metric isδ(z)=√2log1+|z|2∂¯∂log det(g jk)=−ω.Letτ(z)=−n+1n+1δ≤τ≤√n+1.The weighted Bergman space B a p is the space of holomorphic functions g on B suchthatB|g|p(1−|z|)aωn<∞.See[Sto,Ch.10].We have B n2=0.The intersection B n+02= ε>0B n+ε2contains the Hardy space H2(B)of holomorphic functions f on B such that|f|2has a harmonic majorant.11The boundary behaviour of the functions in B n+02may be rather wild.A theorem ofBagemihl,Erd¨o s,and Seidel[BES],[Mac],states that ifµ:[0,1)→[0,∞)goes to inﬁnity at1,then there exists a holomorphic function f on the unit disc with|f|≤µ(|·|),suchthat for some sequence r nր1,we have min|z|=r n|f(z)|→∞.In particular,f does nothave aﬁnite limit along any curve that intersects every neighbourhood of the boundary.Takingµ(r)=−log(1−r),we get f in B1+02.We haveB a2=O2an+1O cτ(B),and deﬁne E(X)similarly.These are Fr´e chet-Hilbert spaces.From now on we let n≥2.Theorem1.2yields the following result.4.1.Theorem.IfΘ>cω,then the restriction mapρ:O cτ(B)→O cτ(X)is an isomorphism.SupposeΘ>2nn+1ω,then X has corona.Proof.Let p∈B\X and f i(z)=z i−p i,i=1,...,n.Then |f i|>ε>0on X.Suppose X has no corona.Then there are g1,...,g n∈H∞(X)with f i g i=1.By Theorem 4.1,g i extends to a function G i∈E(B).Then h= f i G i∈E(B),and h|X=1.Again by Theorem4.1,h=1,which is absurd since h(p)=0.The proof of Theorem2.1shows that ifΘ>6nand similarly for X.In contrast to theﬁrst part of Theorem4.1,we we obtain the following result from Corollary3.5.4.3.Theorem.If s≥1andΘ≤sω,then the restriction mapρ:O cτ(B)→O cτ(X)is not an isomorphism for2n2−n+1c>s+.Furthermore,wen+12ncan easily show thatρis not injective if c>2m+.n+15.A dichotomyAs before,we consider a projective manifold M covered by the unit ball B in C n,n≥2, with a positive line bundle L,and the preimage X in B of an L-curve C in M.We will de-note the covering group byΓ.The bounded extension problem for holomorphic functions is related to the question of which bounded harmonic functions on X are real parts of holo-morphic functions.This question was studied in[L´a r2],where the following dichotomy was established in the more general setting of a nonelementary Gromov-hyperbolic cov-ering space of a compact K¨a hler manifold.5.1.Theorem[L´a r2,Thm.4.2].One of the following holds.(1)Every positive harmonic function on X is the real part of a holomorphic function.(2)If u≥0is the real part of an H1function on X,then the boundary decay of u ata zero on the Martin boundary of X is no faster than its radial decay.By results of Ancona[Anc],the Martin compactiﬁcation of X is naturally homeomor-phic to X∪S,where S=∂B is the unit sphere.13Clearly,if(1)holds,then there are holomorphic functions on X with a bounded real part that do not extend to a holomorphic function on B with a bounded real part.If(1)holds,then each Martin function k p,p∈S,is the real part of a holomorphic function f p on X.Then the holomorphic map exp(−f p):X→D is proper at every boundary point except p.Here,D denotes the unit disc.Also,if p,q∈S,p=q,then the holomorphic map(exp(−f p),exp(−f q)):X→D×D is proper.However,we have the following result.5.2.Theorem.There is no proper holomorphic map X→D.Proof.Bounded holomorphic functions separate points on X,so if there is a proper holomorphic map X→D,then X is Parreau-Widom by a theorem of Hasumi[Has,p. 209].By[L´a r2,Thm.5.1],if X is Parreau-Widom,then X is either isomorphic to D or homeomorphic to the2-sphere with a Cantor set removed.Both possibilities are excluded by the Martin boundary of X being S.When L is suﬃciently ample,we can prove a stronger result.5.3.Theorem.If L is suﬃciently ample and f is a holomorphic function on X,then f−1(U)is not relatively compact in X for any nonempty open subset U of the image f(X).In other words,every value of f is taken at inﬁnity.Proof.Suppose there is a holomorphic function f on X such that f−1(U)is relatively compact in X for some nonempty open subset U of f(X).We may assume that0∈U. Then1/f is a meromorphic function on X which has a pole p and is bounded outside the compact closure of f−1(U).Since bounded holomorphic functions separate points on X, a theorem of Hayashi[Hay]now implies that the natural map from X into the character space of H∞(X)is open when restricted to some neighbourhood of p.By Theorem2.1, this is absurd when L is suﬃciently ample.We will now present another dichotomy in a similar vein.Let C K(S)denote the space of continuous functions S→K,K=R or K=C,with the supremum norm.Let P be the subspace of C R(S)of boundary values of pluriharmonic functions on B which are continuous onB.It is known that if V is a proper closed subspace of C R(S)and V is invariant under the action of the automorphism group G=P U(1,n) of B,then V=R or V=P.Also,if V is a proper closed G-invariant subspace of C C(S), then V is one of the following:C,O,5.4.Theorem.Suppose that the covering groupΓis arithmetic,and that L is a tensor power of the canonical bundle.Then one(and only one)of the following holds.(1)E(C)=P for every L-curve in aﬁnite covering of M.(2)The subspace of C R(S)generated by E(C)for all L-curves C inﬁnite coveringsof M is dense in C R(S).Note that(2)holds if(1)in Theorem5.1holds for the preimage X of some L-curve in aﬁnite covering of M.Proof.Let C be an L-curve in aﬁnite covering M1of M,with preimage X in B.ThenM1=B/Γ1,whereΓ1is a subgroup ofﬁnite index inΓ.Let g be an element of the commensurability subgroup Comm(Γ)in G.This means thatΓand gΓg−1are commen-surable,i.e.,their intersection is ofﬁnite index in both of them.ThenΓ2=Γ1∩gΓ1g−1 is a subgroup ofﬁnite index inΓ1.Ifα∈E(C),so H X[α]=Re f with f holomorphicon X,then f◦g is holomorphic on g−1X and H g−1X[α◦g]=Re f◦g.Ifγ∈Γ2,then γ=g−1γ1g for someγ1∈Γ1,soγg−1X=g−1γ1gg−1X=g−1γ1X=g−1X.Hence,g−1X isΓ2-invariant,so g−1X is the preimage of an L-curve C′in theﬁnitecovering B/Γ2of M(here is where we use the assumption that L is a tensor power of thecanonical bundle),andα◦g∈E(C′).This shows that the subspace E of C R(S)described in(2)is invariant under Comm(Γ). SinceΓis arithmetic,Comm(Γ)is Hausdorﬀ-dense in G[Zim,6.2.4](and in fact con-versely),so the closure E is either P or C R(S),and the theorem follows.If the spaces E(C)are rigid in the sense that they do not change when C is varied in its linear equivalency class,then the theorem yields a strong dichotomy.5.5.Corollary.Suppose thatΓis arithmetic,and that L is a tensor power of the canonical bundle.Suppose also that if C1and C2are L-curves in the sameﬁnite covering of M,then E(C1)=E(C2).Then E(C)is either P or C R(S)for every L-curve C in a ﬁnite covering of M.We obtain analogous results for holomorphic functions.If C is an L-curve in aﬁnite covering of M with preimage X in B,let us denote by F(C)the closed subspace of functionsα∈C C(S)that extend to a holomorphic function on X.Clearly,O⊂F(C), but F(C)is considerably smaller than C C(S).5.6.Lemma.F(C)∩(P+i C R(S))=O.Proof.Letα∈F(C)∩(P+i C R(S)),so H[α]=f∈O(X)and there is u∈C R(B.Now F maps B into a vertical strip.Letσbe an isomorphism from a neighbourhoodof the closure of this strip in the Riemann sphere onto D.Thenσ◦F is a bounded15holomorphic function on B andσ◦F|X=σ◦f,soσ◦F has the same nontangential boundary functionσ◦αasσ◦f.Sinceσ◦αis continuous,σ◦F extends continuously toB)such that(1)u is plurisubharmonic on B,(2)(∂¯∂u)n=0,i.e.,u is maximal,and(3)u|S=α.Let us write u=M[α]=M B[α].This is the solution of the Dirichlet problem for the Monge-Amp`e re operator,due to Bedford and Taylor[BT].See also earlier work of Bremermann[Bre]and Walsh[Wal].In fact,u is given by the Perron-Bremermann formula u=sup Fα,where Fαis the set of all plurisubharmonic functions v on B withv(z)≤α(x),x∈S.lim supz→xThe operator M:C R(S)→C R(ly,ifε>0,thenα−ε≤αi≤α+εfor i suﬃciently large,and then Fα−ε⊂Fαi⊂Fα+ε,so M[α]−ε≤M[αi]≤M[α]+ε.166.1.Theorem.Letα∈C R(S).The following are equivalent.(1)The harmonic extension H[α]ofαto X extends to a bounded-above plurisubhar-monic function on B.(2)H[α]extends to a function in C R(B,so if M[αi]|X are harmonic,then so is M[α]|X.We obtain a dichotomy analogous to those in Section5.If C is an L-curve in aﬁnite covering of M with preimage X in B,let us denote by D(C)the space of functions α∈C R(S)such that M[α]|X is harmonic.Clearly,P⊂D(C)but,as noted above,D(C) is considerably smaller than C R(S).6.3.Theorem.Suppose thatΓis arithmetic,and that L is a tensor power of the canonical bundle.Then one of the following holds.(1)D(C)=P for every L-curve in aﬁnite covering of M.(2)The subspace of C R(S)generated by D(C)for all L-curves C inﬁnite coveringsof M is dense in C R(S).17Suppose furthermore that if C1and C2are L-curves in the sameﬁnite covering of M, then D(C1)=D(C2).Then D(C)=P for every L-curve C in aﬁnite covering of M.There are no examples for which it is known which alternative holds in any of the four dichotomies5.1,5.4,5.7,and6.3,nor is it known if these dichotomies are actually diﬀerent.References[Anc] A.Ancona,Th´e orie du potentiel sur les graphes et les vari´e t´e s,´Ecole d’´e te de probabilit´e s de Saint-Flour XVIII—1988,Lecture Notes in Mathematics,vol.1427,Springer-Verlag,Berlin, 1990,pp.1–112.[BES] F.Bagemihl,P.Erd¨o s,W.Seidel,Sur quelques propri´e t´e s fronti`e res des fonctions holomorphes d´eﬁnies par certains produits dans le cercle-unit´e,Ann.Sci.´Ecole Norm.Sup.(3)70(1953), 135–147.[BD] D.E.Barrett,J.Diller,A new construction of Riemann surfaces with corona,(preprint1996), J.Geometric Analysis(to appear).[BT] E.Bedford,B.A.Taylor,The Dirichlet problem for a complex Monge-Amp`e re equation,Invent.Math.37(1976),1–44.[BO] B.Berndtsson,J.Ortega Cerd`a,On interpolation and sampling in Hilbert spaces of analytic functions,J.reine angew.Math.464(1995),109–128.[Bre]H.J.Bremermann,On a generalized Dirichlet problem for plurisubharmonic functions and pseudo-convex domains.Characterization ofˇSilov boundaries,Trans.Amer.Math.Soc.91 (1959),246–276.[Dem]J.-P.Demailly,Th´e orie de Hodge L2et th´e or`e mes d’annulation,Introduction`a la th´e orie de Hodge,Panoramas et synth`e ses3,Soc.Math.France,1996.[EP]J.Eschmeier,M.Putinar,Spectral decompositions and analytic sheaves,London Math.Society Monographs,new series,vol.10,Oxford University Press,1996.[Gam]T.W.Gamelin,Uniform algebras and Jensen measures,London Math.Society Lecture Note Series,vol.32,Cambridge University Press,1978.[Has]M.Hasumi,Hardy classes on inﬁnitely connected Riemann surfaces,Lecture Notes in Mathe-matics,vol.1027,Springer-Verlag,1983.[Hay]M.Hayashi,The maximal ideal space of the bounded analytic functions on a Riemann surface, J.Math.Soc.Japan39(1987),337–344.[HL]G.Henkin,J.Leiterer,Theory of functions on complex manifolds,Monographs in Mathematics, vol.79,Birkh¨a user,1984.[H¨o r]L.H¨o rmander,Generators for some rings of analytic functions,Bull.Amer.Math.Soc.73 (1967),943–949.[Kol]J.Koll´a r,Shafarevich maps and automorphic forms,Princeton University Press,1995. [Kra]S.G.Krantz,Function theory of several complex variables,2nd ed.,Wadsworth&Brooks/Cole, 1992.[L´a r1] F.L´a russon,An extension theorem for holomorphic functions of slow growth on covering spaces of projective manifolds,J.Geometric Analysis5(1995),281–291.[L´a r2]。

研究生学术英语高原第七单元课文翻译

为什么材料的历史是真正的文化历史？1.每样东西都是由某种东西构成的。

如果把混凝土、玻璃、纺织品、金属和其他材料从我们的生活中拿走，我们就只能赤身裸体，在泥泞的田野里瑟瑟发抖。

我们生活的复杂性在很大程度上是由物质财富赋予的，如果没有我们的文明，我们将很快恢复到动物行为:使我们成为人类的是我们的衣服、我们的家、我们的城市、我们的东西，我们通过我们的习俗和语言赋予这些东西生命。

如果你去过灾区，这一点就会变得非常明显。

然而，物质世界不仅仅是我们技术和文化的展示，它是我们的一部分，我们发明它，我们创造它，它造就了我们。

2.材料的根本重要性从各个文明时代的命名——石器时代、铁器时代和青铜时代——就可以清楚地看出，每个新时代都由一种新材料带来。

钢铁是维多利亚时代的主要材料，工程师们可以充分发挥他们的梦想，建造悬索桥、铁路、蒸汽机和客轮。

Isambard Kingdom Brunel 将其作为改造世界的宣言，并播下现代主义的种子。

20世纪常被誉为硅的时代，在材料科学取得突破后，迎来了硅芯片和信息革命。

然而,其他新材料的万花筒也彻底改变了现代生活。

建筑师将大量生产的平板玻璃与结构钢结合在一起，建造摩天大楼，从而发明了一种新型的城市生活。

塑料改变了我们的家庭和衣着。

聚合物被用来制造电影胶片，并引入了一种新的视觉文化——电影。

铝合金和镍高温合金的发展使我们能够廉价飞行，并加速了文化的碰撞。

医疗陶瓷和牙科陶瓷让我们得以重建自我，重新定义残疾和衰老——正如“整形手术”一词所暗示的那样，材料往往是修复我们的功能(髋关节置换)或增强我们的特征(隆胸硅胶植入物)的新疗法的关键。

3.我对材料的痴迷始于青少年时期。

我对他们的默默无闻感到困惑，尽管他们就在我们身边。

有多少人能看出铝和钢的区别?木头之间明显不同，但有多少人能说出原因?塑料是混杂的;谁知道聚乙烯和聚丙烯的区别?最终，我进入牛津大学(Oxford University)材料科学系攻读学位，接着攻读喷气发动机合金博士学位，现在是伦敦大学学院(University College London)材料与社会教授和制造研究所(Institute of Making)主任。

术语对应的学科名词解释

术语对应的学科名词解释在我们的日常生活和学习中，我们经常会遇到各种各样的术语。

这些术语代表着特定学科中的概念或理论，是学术交流和专业研究的重要工具。

本文将通过一些常见的术语，为读者解释这些术语所对应的学科名词。

1. 熵（Entropy）熵是热力学中的一个重要概念，它代表了一个系统的无序程度。

在物理学中，熵被用来描述能量分布的混乱程度。

而在信息论中，熵则表示信息的不确定性或信息的平均信息量。

因此，熵这个术语既可在物理学中使用，也可在信息科学中使用。

2. 主观性（Subjectivity）主观性是哲学和心理学中常见的概念。

它指的是个体的主观经验和观点，与客观事实相对立。

主观性认为，每个人的认知和感受都是独特而个体化的，因此无法被客观的标准或规则所完全捕捉和测量。

主观性在哲学和心理学领域内有广泛的应用和研究。

3. 多样性（Diversity）多样性是生物学和社会科学领域中的一个重要概念。

在生物学中，多样性描述了一个生态系统或物种群体中的种类和数量多样性。

而在社会科学中，多样性则指代不同文化、民族、性别、背景等方面的差异和多元化。

多样性这个术语在不同学科中的具体定义和应用有所不同，但都强调了不同元素的多样性和丰富性。

4. 可持续发展（Sustainable Development）可持续发展是环境学和经济学中的一个重要概念。

它强调了人类社会的发展需求与生态环境的保护之间的平衡。

可持续发展要求我们在满足当前需求的同时，不损害未来世代的需求和资源。

该概念涉及到经济、社会和环境等多个领域，因此它的学科背景非常广泛。

5. 数据挖掘（Data Mining）数据挖掘是计算机科学和统计学中的一个术语。

它指的是通过从大量数据中提取模式、关联和知识等信息来发现隐藏在数据中的有价值的信息。

数据挖掘可以用于商业、科学和决策等领域，帮助我们了解数据背后的规律和趋势，从而做出更准确的预测和决策。

6. 经典条件作用（Classical Conditioning）经典条件作用是心理学中的一个重要概念，也被称为条件反射。

肿瘤干细胞的研究及进展

具有形成集落的能力
x
Seminar-I
肿瘤干细胞的研究及进展
二、肿瘤干细胞的研究
2.1 肿瘤的形成假说
随机学说(Stochastic)
所有肿瘤细胞均存在无限增殖的可能；但以很小的几率,随机地进入细胞周期增生分裂
等级学说(Hierarchy)
肿瘤细胞在功能上是异质的；只有少量肿瘤干细胞具有无限增殖的可能
8. Al-Hajj M., et al., Oncogene. 23 (2004) 7274.
Seminar-I
肿瘤干细胞的研究及进展
2.5 肿瘤的形成
正常
增殖
增殖↑ 死亡↓ 免疫逃避↑ 自我更新↑
正常干细胞产生多能祖细胞, 继而形成祖细胞和分化细胞，干细胞的突变可以引起异常增殖而形成癌前病变，附加突变使其获得无限增殖, 凋亡缺失, 免疫逃避, 进一步生长为恶性肿瘤.
Thank you !
Your comments are welcomed !
Seminar-I
肿瘤干细胞的研究及进展
肿瘤干细胞研究的意义
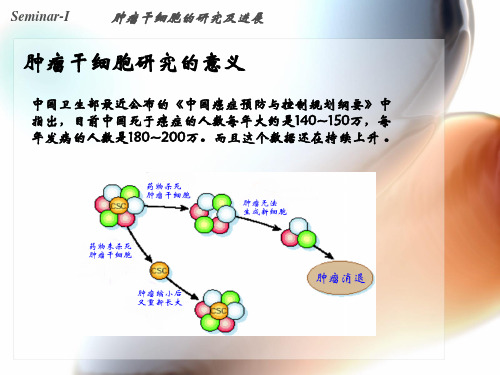
中国卫生部最近公布的《中国癌症预防与控制规划纲要》中指出，目前中国死于癌症的人数每年大约是140~150万，每年发病的人数是180~200万。而且这个数据还在持续上升。
Seminar-I
肿瘤干细胞的研究及进展
一、研究背景
干细胞
干细胞
干细胞
干细胞高表达抗药蛋白1 (BCRP1)能够将药物泵出细胞外的特性,用荧光染料 Hoechest 33342对肿瘤细胞进行预染,然后用流式细胞仪进行分选
7. Setoguchi T., et al., Cell cycle 3 (2004) 414.

托福阅读TPO25(试题+答案+译文)第1篇-ThesurfaceofMars

托福阅读TPO25(试题+答案+译文)第1篇:ThesurfaceofMarsTPO是我们常用的托福模考工具，对我们的备考很有价值，下面我给大家带来托福阅读TPO25(试题+答案+译文)第1篇:The surface of Mars。

托福阅读原文【1】The surface of Mars shows a wide range of geologic features, including huge volcanoes-the largest known in the solar system-and extensive impact cratering. Three very large volcanoes are found on the Tharsis bulge, an enormous geologic area near Mars’s equator. Northwest of Tharsis is the largest volcano of all: Olympus Mons, with a height of 25 kilometers and measuring some 700 kilometers in diameter at its base. The three large volcanoes on the Tharsis bulge are a little smaller-a “mere”18 kilometers high.【2】None of these volcanoes was formed as a result of collisions between plates of the Martian crust-there is no plate motion on Mars. Instead, they are shield volcanoes — volcanoes with broad, sloping slides formed by molten rock. All four show distinctive lava channels and other flow features similar to those found on shield volcanoes on Earth. Images of the Martian surface reveal many hundreds of volcanoes. Most of the largest volcanoes are associated with the Tharsis bulge, but many smaller ones are found in the northern plains.【3】The great height of Martian volcanoes is a direct consequence of the planet’s low surface gravity. As lava flows and spreads to form a shield volcano, the volcano’s eventual height depends on the new mountain’s ability to support its own weight. The lower the gravity, the lesser the weight and the greater the height of the mountain. It is no accident that Maxwell Mons on Venus and the Hawaiian shield volcanoes on Earth rise to about the same height (about 10 kilometers) above their respective bases-Earth and Venus have similar surface gravity. Mars’s surface gravity is only 40 percent that of Earth, so volcanoes rise roughly 2.5 times as high. Are the Martian shield volcanoes still active? Scientists have no direct evidence for recent or ongoing eruptions, but if these volcanoes were active as recently as 100 million years ago (an estimate of the time of last eruption based on the extent of impact cratering on their slopes), some of them may still be at least intermittently active. Millions of years, though, may pass between eruptions.【4】Another prominent feature of Mars’s surface is cratering. The Mariner spacecraft found that the surface of Mars, as well as that of its two moons, is pitted with impact craters formed by meteoroids falling in from space. As on our Moon, the smaller craters are often filled with surface matter-mostly dust-confirming that Mars is a dry desert world. However, Martian craters get filled in considerably faster than their lunar counterparts. On the Moon, ancient craters less than 100 meters across (corresponding to depths of about 20 meters) have been obliterated, primarily by meteoritic erosion. On Mars, there are relatively few craters less than 5 kilometers in diameter. The Martian atmosphere is an efficient erosive agent, with Martian winds transporting dust from place to place and erasing surface features much faster than meteoritic impacts alone can obliterate them.【5】As on the Moon, the extent of large impact cratering (i.e. craters too big to have been filled in by erosion since they were formed) serves as an age indicator forthe Martian surface. Age estimates ranging from four billion years for Mars’s southern highlands to a few hundred million years in the youngest volcanic areas were obtained in this way.【6】The detailed appearance of Martian impact craters provides an important piece of information about conditions just below the planet’s surface. Martian craters are surrounded by ejecta (debris formed as a result of an impact) that looks quite different from its lunar counterparts. A comparison of the Copernicus crater on the Moon with the (fairly typical) crater Yuty on Mars demonstrates the differences. The ejecta surrounding the lunar crater is just what one would expect from an explosion ejecting a large volume of dust, soil, and boulders. However, the ejecta on Mars gives the distinct impression of a liquid that has splashed or flowed out of crater. Geologists think that this fluidized ejecta crater indicates that a layer of permafrost, or water ice, lies just a few meters under the surface. Explosive impacts heated and liquefied the ice, resulting in the fluid appearance of the ejecta.托福阅读试题1.The word “enormous”(paragraph 1)in the passage is closest in meaning toA.importantB.extremely largeC.highly unusualD.active2.According to paragraph 1, Olympus Mons differs from volcanoes on the Tharsis bulge in that Olympus MonsA.has more complex geologic featuresB.shows less impact crateringC.is tallerD.was formed at a later time3.The word “distinctive”(paragraph 1)in the passage is closest in meaning toA.deep.plex.C.characteristic.D.ancient.4.According to paragraphs 1 and 2, which of the following is NOT true of the shield volcanoes on the Tharsis bulge?A.They have broad, sloping sides.B.They are smaller than the largest volcano on Mars.C.They have channels that resemble the lava channels of volcanoes on Earth.D.They are over 25 kilometers tall.5.The word “roughly” in the passage is closest in meaning toA.typically.B.frequently.C.actually.D.approximately.6.In paragraph 3, why does the author compare Maxwell Mons on Venus to the Hawaiian shield volcanoes on Earth?A.To help explain the relationship between surface gravity and volcano height.B.To explain why Mars’s surface gravity is only 40 percent of Earth’s.C.To point out differences between the surface gravity of Earth and the surface gravity of Venus.D.To argue that there are more similarities than differences between volcanoes on different planets.7.Which of the sentences below best expresses the essential information in the highlighted sentence in the passage? Incorrect choices change the meaningin important ways or leave out essential information.A.Although direct evidence of recent eruptions is lacking, scientists believe that these volcanoes were active as recently as 100 million years ago.B.Scientists estimate that volcanoes active more recently than 100 years ago will still have extensive impact cratering on their slopes.C.If, as some evidence suggests, these volcanoes erupted as recently as 100 million years ago, they may continue to be intermittently active.D.Although these volcanoes were active as recently as 100 million years ago, there is no direct evidence of recent or ongoing eruptions.8.The word “considerably”(paragraph 3)in the passage is closest in meaning toA.frequently.B.significantly.C.clearly.D.surprisingly.9.According to paragraph 4, what is demonstrated by the fact that cratersfill in much faster on Mars than on the Moon?A.Erosion from meteoritic impacts takes place more quickly on Mars than on the Moon.B.There is more dust on Mars than on the Moon.C.The surface of Mars is a dry desert.D.Wind is a powerful eroding force on Mars.10.In paragraph 4, why does the author point out that Mars has few ancient craters that are less than 5 kilometers in diameter?A.To explain why scientists believe that the surface matter filling Martian craters is mostly dust.B.To explain why scientists believe that the impact craters on Mars were created by meteoroids.C.To support the claim that the Martian atmosphere is an efficient erosive agent.D.To argue that Mars experienced fewer ancient impacts than the Moon did.11.According to paragraph 5, what have scientists been able to determinefrom studies of large impact cratering on Mars?A.Some Martian volcanoes are much older than was once thought.B.The age of Mars’s surface can vary from area to area.rge impact craters are not reliable indicators of age in areas with high volcanic activity.D.Some areas of the Martian surface appear to be older than they actually are.12.According to paragraph 6, the ejecta of Mars’s crater Yuty differs fromthe ejecta of the Moon’s Copernicus crater in that the ejecta of the Yuty craterA.Has now become part of a permafrost layer.B.Contains a large volume of dust, soil and boulders.C.Suggests that liquid once came out of the surface at the crater site.D.Was thrown a comparatively long distance from the center of the crater.13. Look at the four squares【■】that indicate where the following sentence could be added to the passage.Where would the sentence best fit? Click on a square to add the sentence to the passage. This surface feature has led to speculation about what may lie under Mars’s surface.The detailed appearance of Martian impact craters provides an important pieceof information about conditions just below the planet’s surface. Martian craters are surrounded by ejecta (debris formed as a result of an impact) that looks quite different from its lunar counterparts. A comparison of the Copernicus crater on the Moon with the (fairly typical) crater Yuty on Mars demonstrates the differences. The ejecta surrounding the lunar crater is just what one would expect from an explosion ejecting a large volume of dust, soil, and boulders. ■【A】However, the ejecta on Mars gives the distinct impression of a liquid that has splashed or flowed out of crater. ■【B】Geologists think that this fluidized ejecta crater indicates that a layer of permafrost,or water ice, lies just a few meters under the surface. ■【C】Explosive impacts heated and liquefied the ice, resulting in the fluid appearance of the ejecta. ■【D】14. Directions: An introductory sentence for a brief summary of the passage is provided below. Complete the summary by selecting the THREE answer choices that express the most important ideas in the passage. Some sentences do not belong in the summary because they express ideas that are not presented in the passage or are minor ideas in the passage. This question is worth 2 points.Drag your answer choices to the spaces where they belong. To remove an answer choice, click on it. To review the passage, click VIEW NEXT.Volcanoes and impact craters are major features of Martiangeology.A.Plate motion on Mars, once considered to have played no role in shaping the planet’s surface, is now seen as being directly associated with the planet’s earliest volcanoes.B.Mars has shield volcanoes, some of which are extremely tall because of the planet’s low surface gravity.C.Although the erosive power of the Martian atmosphere ensures that Mars has fewer craters than the Moon does, impact craters are prominent on Mars’ s surface.D.Scientists cannot yet reliably estimate the age of the Martian surface because there has been too much erosion of it.E.Scientists have been surprised to discover that conditions just below the surface of Mars are very similar to conditions just below the surface of the MoonF.Studies of crater ejecta have revealed the possibility of a layer of permafrost below the surface of Mars.托福〔阅读答案〕1.enormous 巨大的，所以正确答案是B，extremely large。

On certain quasi-local spin-angular momentum expressions for large spheres near the null in

a r X i v :g r -q c /0109047v 1 13 S e p 2001On certain quasi-local spin-angular momentum expressions for large spheres near the null inﬁnityL´a szl´o B.SzabadosResearch Institute for Particle and Nuclear PhysicsH-1525Budapest 114,P.O.Box 49,HungaryE-mail:lbszab@rmki.kfki.huThe recently suggested quasi-local spin-angular momentum expressions,based on the Bramson superpotential and on the holomorphic or anti-holomorphic spinor ﬁelds,are calculated for large spheres near the future null inﬁnity of asymptotically ﬂat Einstein–Maxwell spacetimes.It is shown that although the expression based on the anti-holomorphic spinors is ﬁnite and unambiguously deﬁned only in the center-of-mass frame (i.e.it diverges in general),the corresponding Pauli–Lubanski spin is always ﬁnite,free of gauge ambiguities,and is built only from the gravitational data.Thus it deﬁnes a gravitational spin expression at the future null inﬁnity.The construction based on the holomorphic spinors diverges in presence of outgoing gravitational radiation.For stationary spacetimes both constructions reduce to the ‘standard’expression.1.Introduction The energy-momentum and angular momentum density of matter ﬁelds is described by their energy-mom-entum tensor T ab ,whose contraction with a Killing ﬁeld K a gives a divergence free current T ab K b .The ﬂux of this current on a compact,spacelike hypersurface Σwith boundary S :=∂Σis therefore conserved,i.e.depends only on the boundary S and independent of the rest of the hypersurface.In Minkowski spacetime the independent Killing 1-forms are the translations K a e :=∇e x a and the rotations K ab e :=x a ∇e x b −x b ∇e x a ,where x a are the standard Cartesian coordinates,a ,b =0,...,3.The corresponding conserved quantities are P a S := ΣT ab t a K a b dΣand J ab S := ΣT ab t a K ab b dΣ,where t a is the future directed unit timelike normal to Σand dΣis the induced volume element on Σ.P a S and J ab S can be interpreted as the quasi-local energy-momentum and angular momentum of the matter ﬁelds associated with the 2-surface S ,respectively.If Σextends to the spacelike inﬁnity i 0or to the future (or past)null inﬁnity I ±of the Minkowski spacetime,then,provided the limits exists (by imposing certain fall-oﬀand global integral conditions,e.g.parity conditions at the spacelike inﬁnity),then they deﬁne the global energy-momentum and angular momentum.In general relativity the usual fall-oﬀconditions on the 3-metric and extrinsic curvature on a spacelike hypersurface ensure that the ADM energy and spatial momentum are ﬁnite and unambiguously deﬁned[1,2].However,to have ﬁnite and unambiguously deﬁned angular momentum and center-of-mass additional conditions,e.g.explicit parity conditions on the three-metric h ab and extrinsic curvature χab ,have to be imposed [2,3].These are preserved by the evolution equations,ensure the functional diﬀerentiability of the Hamiltonian on the whole phase space,and yield unique Poincare structure both for the lapse-shift at inﬁnity and the Hamiltonian.At spatial inﬁnity the energy-momentum and relativistic angular momentum are the value of the functionally diﬀerentiable Hamiltonian,which are just the familiar ADM energy-momentum andspatial angular momentum,and the center-of-mass of Beig and ´OMurchadha [3].Thus,although there are interesting open issues,e.g.whether the angular momentum measured at the spacelike inﬁnity enters the Penrose inequality or not [4],the spatial inﬁnity case is well understood.At null inﬁnity there is a generally accepted deﬁnition of the energy-momentum,which is the Bondi–Sachs energy-momentum.However,on the deﬁnition of angular momentum there is no consensus at all, and there are various suggestions for that:The constructions based on the Komar–Winicour–Tamburino expression are intended to be associated with any BMS vectorﬁeld,and,in the special case of the boost-rotation vectorﬁelds,they can be interpreted as angular momentum[5-7].The suggestion of Ashtekar and Streubel is based on symplectic techniques[8],which turned out later to be connected with the Winicour–Tamburino linkages[9].The general form of other expressions are based on charge integrals of the curvature [10-16]:In particular,although the suggestion of Penrose[12]is based on the solutions of the2-surface twistor equations and the concept of‘origin’is largely decoupled from the cuts of I+,itﬁts nicely to the symplectic structure of I+[17-20].Other remarkable suggestion in this class is due to Moreschi[13-16].He considers(and,together with Dain,prove[15]the existence of)a foliation of I+by special cuts(the‘nice cuts’),intended to model the‘system of rest frames’.Thus he is able to deﬁne not only a(supertranslation–ambiguity–free)angular momentum,but higher order moments of the‘gravitationalﬁeld’,too.In their classic paper Bergmann and Thomson[21]raises the idea that while the energy-momentum of gravity is connected with the spacetime diﬀeomorphisms,the angular momentum should be connected with its internal O(1,3)-symmetry.Thus the angular momentum should be analogous with the spin.This idea was formulated mathematically by Bramson[22-24],whose speciﬁc angular momentum expression was based on the superpotential derived from Hilbert’s Lagrangian and the solutions of the asymptotic twistor equations. Recently,Katz and Lerer[25]could recover the Bondi–Sachs energy-momentum from the standard Noether analysis using asymptoticallyﬂat backgrounds,and suggested an expression for the angular momentum and center-of-mass.Interestingly enough,although these suggestions are based on diﬀerent ideas and yield mathematically inequivalent deﬁnitions,all these resulting expressions bear some resemblance to each other. It is not clear whether the recent suggestion by Rizzi[26],based on a special foliation of the spacetime near the null inﬁnity and reducing to the ADM angular momentum at the spatial inﬁnity[27],also takes such a form.At the quasi-local level the situation is even worse.Although the general framework of how the various quasi-local quantities should look like is more or less well understood(see e.g.[28]),only a few explicit deﬁnitions have been suggested for the energy-momentum and angular momentum.Theﬁrst such suggestion, due to Penrose[12],is,however,not complete:In addition to the(symmetric)kinematical twistor Aαβtwo additional twistors,a Hermitian metric twistor hαβ′and the analog of the(skew,simple)inﬁnity twistor Iαβ, would be needed to reduce the ten complex components of Aαβ(the quasi-local quantities)to ten real ones, and to isolate four of them as the energy-momentum and six of them as the angular momentum.Although at the future null inﬁnity these extra twistors exist and the construction works properly,reproducing the Bondi–Sachs energy-momentum and gives a deﬁnition for the angular momentum,it is not clear whether the additional twistors exist and the construction is viable quasi-locally or not.In principle the Brown–York approach also can yield both energy-momentum and angular momentum[29,30].However,instead of a4-covariant energy-momentum this approach yields separated energy and linear momentum,and it is not a priori clear whether they form a single Lorentz-covariant object.Furthermore,in lack of a unique prescription how the reference conﬁguration should beﬁnd(imbedding of the2-surface into a3-plane[29], or into the light cone in Minkowski spacetime[31,32],or restrict the imbedding in some other way[33]),this construction is not complete either.To deﬁne Lorentz-covariant energy-momentum and angular momentum Ludvigsen and Vickers[34]used the Nester–Witten and the Bramson superpotentials,respectively,but their choice for the two spinorﬁelds in the superpotential depends on the asymptotic structure of the spacetime. Thus their deﬁnition is not genuinely quasi-local.Genuinely quasi-local,manifestly Lorentz-covariant energy momenta,based on the Nester–Witten2-form and either the holomorphic or the anti-holomorphic spinor ﬁelds,were suggested by Dougan and Mason[35],but they did not give any speciﬁc deﬁnition for theangular momentum.Recently we suggested to complete the Dougan–Mason energy-momenta by a spin-angular momentum expression based on Bramson’s superpotential,but,instead of the Ludvigsen–Vickers prescription for the spinorﬁelds,we suggested to use holomorphic or anti-holomorphic spinorﬁelds[36]. These have already been studied in various situations(pp-waves[36]and small spheres[37]).In the present paper these spin-angular momentum expressions will be calculated for large spheres near the future null inﬁnity.In[22]Bramson introduced his superpotential as the superpotential for the conserved O(1,3)-current C a ab,derived from Hilbert’s second order Lagrangian(considering that as a function of the tetradﬁeld and the O(1,3)-connection1-forms).However,he deﬁned C a ab as the partial derivative of Hilbert’s action with respect to the connection1-forms,while in gauge theories the conserved currents corresponding to the internal gauge invariance are the variational derivatives of the particle action with respect to the connection 1-forms.In fact,this variational derivative is zero.Thus,in Section2,ﬁrst we show that Bramson’s superpotential can be derived from Møller’s tetrad action in a correct way,and then we review the status of our speciﬁc quasi-local spin-angular momentum expressions and provide the general formulae for the large sphere calculations.In Section3.we review those structures of the future null inﬁnity that we need, especially the BMS translations and rotations and their spinor form,and quote the asymptotic solution of the Einstein–Maxwell equations from[38].Then,in Section4,we calculate the anti-holomorphic spin-angular momentum for large spheres expanding that as a series of r−1.We will see that although this is diverging linearly and itsﬁnite order part is ambiguous in general,the Pauli–Lubanski spin vector built from the anti-holomorphic Dougan–Mason energy-momentum and spin-angular momentum is alwaysﬁnite and well deﬁned.To demonstrate this,we need the O(r−1)accurate expansion of the Dougan–Mason energy-momentum.Since,however,this calculation has been done only for stationary spacetimes[39](and apparently an r−1order term was overlooked),we have to clarify the asymptotic behaviour of the energy-momentum,too.In Section5we show that in stationary spacetimes the anti-holomorphic construction reduces to the‘standard’expression.The holomorphic construction,calculated in Section6,is diverging quadratically in presence of outgoing gravitational radiation,but gives the same‘standard’expression for stationary spacetimes.Finally,in the Appendix we discuss how the various spinor equations determine special spin frames on two-spheres,in particular,on round spheres and on large spheres near the future null inﬁnity.We show how the BMS translations and rotations can be recovered from them.We found that, in addition to the usual representations,they can also be recovered from the solutions of the limit of the Dirac–Witten equations on the unit sphere cuts of I+,too.We treat the2-surface twistor equations also in the traditional way instead of the almost exclusively followed conformal method.Our conventions and notations are mostly those of[40].In particular,we use the abstract index notations,and only the underlined and boldface indices take numerical values.The signature is-2,the Riemann-and Ricci tensors and the curvature scalar are deﬁned by−R a bcd X b Y c Z d:=∇Y(∇Z X a)−∇Z(∇Y X a)−∇[Y,Z]X a,R ab:=R e aeb and R:=R ab g ab,respectively.Thus Einstein’s equations take the form R ab−116πG16πGC a ab:=4α|g|δI U4πG∇bE a a E b b−E a b E b a ,(2.1)whereαis some normalization constant,to be determined in some special situation.C a ab is identically con-served,and we call the corresponding superpotential2-form,W ac ac:=12εab cd=−α1|g|C a a:=δI U/δE a a gives the tensorial energy-momentum expression t b a for the gravitational‘ﬁeld’found in[42,43](and see also[44]): C a a=ϑa b t b a.)If S is any closed orientable spacelike2-surface in M andλA A,A=0,1,is a pair of smooth spinorﬁelds on S such that they form a normalized spin-frame,i.e.εABλA AλB B=εAB,whereεAB is the antisymmetric Levi-Civita symbol,thenϑa a:=σa AB′λA A¯λB′A′is an orthonormal1-formﬁeld on S,whereσa AB′are the standard SL(2,C)Pauli matrices.Since the Bramson superpotential depends onϑa a algebraically,the integral J ab can be expressed in terms of the normalized spin frameﬁelds deﬁned only on S.J ab is independent of the extension of{λA A}oﬀthe2-surface S.Translating the tensor name indices of J ab into spinor name indices, and deﬁning its anti-self-dual part by J AA′BB′=:εAB¯J A′B′+εA′B′J AB,weﬁnd thatJ AB=iα8πGS¯λB′A′∇BB′λA A−¯λB′B′∇AA′λA B .(2.3)For any pair of spinorﬁeldsλA A this deﬁnes a Lorentzian4-vector,and it is natural to choose the spinor ﬁelds in(2.2)and(2.3)to be the same.(A more detailed discussion of these issues,and,in particular,the connection of these concepts and the current C a a will be given elsewhere[45].)The quasi-local Pauli–Lubanski spin vector will be deﬁned in the standard way byS AA′:=1mS AA′.)To complete the construction of these quantities,however, the spin frameﬁeldλA A must be speciﬁed on,and only on S.In the present paper we will assume that the spin frame is holomorphic,¯m e∇eλA=0,or anti-holomorphic,m e∇eλA=0.Here m a and¯m a are the standard complex null vectors tangent to S and normalized by¯m a m a=−1,by means of which the metric area-element on S is−i m[a¯m b].In fact,one can show that in the generic case there are two holomorphic/anti-holomorphic spinorﬁelds and they can be normalized,provided S is homeomorphic to S2.Thus P AB′and J AB that we will study here are the two energy-momenta of Dougan and Mason[35],and the two spin-angular momenta that we introduced in[36],respectively.Then the formers can also be written as P AB′=−γi:={o A,ιA},A A:={−ιA,o A}is its dual basis and the spinor components with respect to this frame are deﬁned byλA A=:εA,then the condition of holomorphy can be written as′∂′λA1+σ′λA0=0and′∂′λA0+ρλA1=0;and the condition of anti-holomorphy is equivalent to′∂λA0+σλA1=0and′∂λA1+ρ′λA0=0.Thus boldface capital indices are referring to a basis in the space of solutions,while the underlined capital indices to the GHP spin frame.For example,for a round sphere[39]of radius r the two linearly independent anti-holomorphic and holomorphic spinorﬁelds are given by(A.2.2)and(A.3.2)of the Appendix,respectively.Substituting them into(2.2)we get zero,as could be expected in a spherically symmetric system.It is known that the anti-holomorphic Dougan–Mason energy-momentum is a future directed non-spacelike vector if S is the boundary of some compact spacelike hypersurfaceΣ,the matterﬁelds satisfy the dominant energy condition onΣ,and S is convex in the weak sense thatρ′≥0[35].(For the holomorphic construction the analogous convexity condition isρ≤0.)Furthermore,if the dominant energy condition holds on the whole domain of dependence D(Σ)ofΣthen P AB′is vanishing if and only if D(Σ)isﬂat, and this is also equivalent to the existence of two constant spinorﬁelds on S with respect to the covariant derivative∆e(see the Appendix);and P AB′is null if and only if D(Σ)has a pp-wave metric and the matter is pure radiation,which is also equivalent to the existence of one constant spinorﬁeld on S[46,47].J AB has already been calculated for(axi-symmetric)S bounding a pp-wave Cauchy development and it was shown that the Pauli–Lubanski spin S AB′is proportional to the(null)P AB′[36].We have already calculated(2.2)for the Ludvigsen–Vickers-,the holomorphic-and the anti-holomorphic spinors for small spheres S r of radius r with respect to an observer t a at a point o∈M[37].Considering this to be a function of the radius,J AB,it can be expanded as a power series of r.The leading term isr−4αr6T AA′BB′CC′DD′t AA′t BB′45Gt CC′t D′EεDF E(A E E B)F in all cases,where T abcd is the Bel–Robinson tensor and{E A A,E A A}is the dual Cartesianspin frame at o;i.e.E a a=σAA′a E A A¯E A′A′andϑa a=σa AA′E A A¯E A′A′form an orthonormal dual frame at o.Thus the‘pure gravitationalﬁeld’itself does not seem to contribute to the spin-angular momentum in r4order. On the other hand,for the leading term in the similar expansion of the quasi-local(anti-self-dual)angular momentum of the matterﬁelds in Minkowski spacetime we get4by J ABr.Then,according to the general philosophy of the large sphere calculations[38,39],we expand thespinor componentsλA Ar.The expansion coeﬃcients as functions of the remaining coordinates depend on the actual construction(holomorphic or anti-holomorphic).If thereforeλA A(0)+1(1)+1(2)+...and d S r=:r2(1+1r2s(2)+...)d S, where d S is the area element on the unit sphere S,then(2.2)takes the form*J AB r =1rs(1)+14πGSr ρ′(1)λA0(0)¯λB′0′(0)+ρ(1)λA1(0)¯λB′1′(0) ++ ρ′(1) λA0(0)¯λB′0′(1)+λA0(1)¯λB′0′(0) +ρ′(2)λA0(0)¯λB′0′(0)+ρ(1) λA1(0)¯λB′1′(1)++λA1(1)¯λB′1′(0) +ρ(2)λA1(0)¯λB′1′(0) ++1rs(1)+1*We use two diﬀerent notations for the expansion coeﬃcients:f(k)(i.e.when the index k is between parentheses)denotes the coeﬃcient of r−k in the expansion,which may turn out later to be zero.On the other hand,as is usual in the relevant literature,f k will denote the(k+1)th nonvanishing expansion coeﬃcient of the function f=f(1whereδ:=m a∇a=:P(∂/∂¯ζ)+Q(∂/∂ζ),andζ,¯ζare the standard complex stereographic coordinates on the 2-sphere.In particular,on the unit sphere in the Minkowski spacetime the edth and edth-prime operatorstake the form0′∂f:=12(1+ζ¯ζ)(∂f/∂¯ζ)+12(p−q)ζf and0′∂′f:=12(1+ζ¯ζ)(∂f/∂ζ)−12(p−q)¯ζf,which will be used in the subsequent calculations.Overdot will denote partial derivative with respect to u.In the coordinate system(u,w,ζ,¯ζ),where w:=r−1and the future null inﬁnity is given by w=0, the BMS vectorﬁelds have the form k a=(H+(c i+¯c i)t i u)(∂∂ζ)a+¯c i¯ξi(∂∂u)a+O(r−1),a=0,...,3,where t0:=1and t i for i=1,2,3are given explicitly above.The functionst a can be written as t a=σAB′a τA¯τB′,whereτ0:=exp(iα)4√2andτ1:=−exp(iα)4√2,and exp(iα)is an unspeciﬁed phase.In Minkowski spacetime the standard constant orthonormal frameﬁeld {E a a}(i.e.the translational Killing vectors K a a)has precisely this asymptotic form,and if the phase exp(iα) is chosen to be−i then the functionsτA are just E A A o A,the contractions of the Cartesian spin frame with the GHP spin vector o A.In fact,in terms of the GHP spin frameεA A4√ 2ιA ,I A=−i211+ζ¯ζ o A−√√2εi jk K a jk),where i,j,k=1,2,3andσA i B:=√√∂u)a+ξi(∂√√r+1√r2σ0+16ψ00−(σ0)2¯σ0 +O(r−5).(3.3)This implies that the area element of the large sphere of radius r is d S (u,r )=r 2(1−r −2σ0¯σ0+O (r −4))d S ;i.e.in equation (2.4)s (1)=0and s (2)=−σ0¯σ0.The spin coeﬃcients with deﬁnite (p,q )type areσ=1r−1r ˙¯σ0−12¯σ0−0′∂′0′∂¯σ0 −12¯σ0ψ20+12r +1r 3 0′∂¯σ00′∂′σ0−120′∂′ψ10+1r 20′∂′σ0−1r 12ζ−12√r 3 12ζσ0¯σ0+12(e +i µ).Here M ,e and µare real constants,interpreted as the total mass,the total electric charge and the total magnetic charge,respectively,and J is a real function with structure J = m =1m =−1J m Y 1,m for some constants J 0and J ±1,where Y 1,m are the standard j =1spherical harmonics.Rewriting J by the familiar polar coordinates (θ,φ)(deﬁned by ζ=:cot θ√2+i φ.)4.The anti-holomorphic spin-angular momentumSubstituting the expansion λA (0)+1(1)+1(2)+...of the spinor components and the expressions(3.2-9)for the functions P and Q and the spin coeﬃcients into the equations deﬁning the anti-holomorphic spinor ﬁelds on S (u,r ),we obtain the following hierarchy of equations′∂λ1(0)+12λ0(1)=− ψ20+σ0˙¯σ0+0′∂2¯σ0 λ0(0),(4.2.a) 0′∂λ0(1)=0,(4.2.b)0′∂λ1(2)+12ψ10 λ1(0)++ 0′∂¯σ00′∂′σ0+σ00′∂′0′∂¯σ0+¯σ00′∂0′∂′σ0+120′∂¯ψ1′0−2Gφ10¯φ1′0 λ0(0),(4.3.a)′∂λ0(2)=σ0 0′∂′λ0(1)−λ1(1) − σ00′∂¯σ0+1(0),A=0,1,of(4.1.a-b),and we choose them to be given explicitly by(A.2.2)withρ′=1(1)→λA(0)andλA(2)+D AλA A2λB0(1) +2λB1(0) 0′∂λA1(1)+14πGSψ20+σ0˙¯σ0 λA1(0)λB0(0)+λA0(0)λB1(0) d S(4.5)is vanishing.(We return to the discussion of L AB below.)Next let us consider the r0order term of(2.5):12λA 0(2)λB 1(0)−− 0′∂λB 1(0) λA 1(2)+λA 1(1) −0′∂λB 1(1)−(ψ00+σ0˙¯σ0+0′∂2¯σ0)λB 0(0) +12σ0¯σ0 λA 0(0)λB 1(0)+λA 1(0)λB 0(0) d S == SλA 1(0) 0′∂λB 1(2)+12σ0¯σ0λB 0(0) +λB 1(0) 0′∂λA 1(2)+12σ0¯σ0λA 0(0) −− ψ00+σ0˙¯σ0+0′∂2¯σ0 λA 0(0)λB 1(1)+λA 1(1)λB 0(0) d S == S −ρ′(3) λA 0(0)λB 1(0)+λA 1(0)λB 0(0) ++ ψ10+2σ00′∂¯σ0 λA 1(0)λB 1(0)+σ0 λA 1(0)0′∂′λB 1(1)+λB 1(0)0′∂′λA 1(1) −− ψ20+σ0˙¯σ0+0′∂2¯σ0 λA 0(0)λB 1(1)+λA 0(1)λB 1(0)+λA 1(0)λB 0(1)+λA 1(1)λB 0(0) d S ,(4.6)where ρ′(3)is the 3rd order term of ρ′in (3.7),and we used (4.1.a),(4.2.a)and (4.3.a).Substituting (3.7)here,using (4.1.b)and the consequences 0′∂′λA 1(0)=0and 0′∂′λA 0(0)=λA 1(0)of (4.1),the integral of the ﬁrst three terms of the right hand side of (4.6)can be written asSψ10+2σ00′∂¯σ0 λA 1(0)λB 1(0)−0′∂′σ0 λA 1(0)λB 1(1)+λB 1(0)λA 1(1) + 0′∂¯σ00′∂′σ0−120′∂′ψ10+12¯ψ1′0+¯σ00′∂′σ0 λA 0(0)λB 0(0)−0′∂′σ0 λA 1(0)λB 1(1)+λB 1(0)λA 1(1) −− 0′∂′σ00′∂¯σ0+12 S ¯ψ1′0+2¯σ00′∂′σ0+0′∂′(σ0¯σ0) λA 0(0)λB 0(0)−4G 0′∂′(φ10¯φ1′0) λA 0(0)λB 1(0)+λA 1(0)λB 0(0) −−20′∂′σ0 λA 1(0)(λB 1(1)+λB 0(0)0′∂¯σ0)+λB 1(0)(λA 1(1)+λA 0(0)′∂¯σ0) d S .(4.7)Using (4.2.a)again,the integral of the last term on the right hand side of (4.6)can be rewritten as− S ψ20+σ0˙¯σ0+0′∂2¯σ0 λA 0(0)λB 1(1)+λA 0(1)λB 1(0)+λA 1(0)λB 0(1)+λA 1(1)λB 0(0) d S == S λB 1(1) 0′∂λA 1(1)+12λB0(1) −− ψ20+σ0˙¯σ0+0′∂2¯σ0 λA 0(1)λB 1(0)+λA 1(0)λB 0(1) d S == S λA 0(1) 12λA 1(1)− ψ20+σ0˙¯σ0+0′∂2¯σ0 λA 1(0) d S .(4.8)Therefore,λA0(2)andλA1(2)are not needed to calculate the anti-holomorphic spin-angular momentum,but λA0(1)andλA1(1)do appear in(4.6)explicitly.Since,however,the physical quantities should not be sensitive to the addition of the spurious zeroth order solutions toλA0(1)andλA1(1),and by such solutionsλA0(1)=0can always be achieved,whenever(4.8)gives zero,that should be a gauge term.To see that,in fact,this is the case,recall that(4.1.b)and(4.2.b)are the same,thus we may writeλA0(1)=C A CλC0(0)=−2C A C0′∂λC1(0) for some constant complex2×2matrix C A C.(However,C A B is not quite arbitrary,that is restricted by the requirement that the pair of anti-holomorphic spinorﬁelds should form a normalized spin frame.In fact,fromεAB=εA(λA ArλA A(0)+1(1)+...)it follows that C AB must be symmetric.) Substituting this into(4.8)and using(4.2.a)we obtain−2 C A CδB D+C B CδA D S0′∂λC1(0) 12λD0(1)−20′∂ ψ20+σ0˙¯σ0+0′∂2¯σ0 λD1(0) d S= =12πSφ10¯φ1′0 λA0(0)λB1(0)+λA1(0)λB1(0) d S,(4.11)I AB:=1emphasizing that these generators of the anti-self-dual rotation BMS vectorﬁelds emerged naturally,like the approximate rotation-boost Killing vectors in the small sphere calculations[37],without putting them into the general formulae by hand.Although I AB depends on the solutionλA1(1)of(4.2.a)(and hence its integrand is a genuinely non-local expression),that is independent of the gauge solutions.In fact,using′∂′λA1(0)=0,it is easy to see that the addition of a gauge solution toλA1(1)changes the integrand by a total′∂′-derivative.To clarify the meaning of the term of the integrand involvingλA1(1),recall that the BMS vectorﬁelds k a AB are tangent only to the origin cut,and they can be represented completely byλA0(0)λB0(0) only there.On general cuts k a AB contain correction terms proportional to the generator(∂4πGSψ20+σ0˙¯σ0 λA0(0)¯λB′0′(0)d S.(4.13)To see this,let us substitute the explicit solutions(A.2.2)into(4.5).We obtain L00=−√√2∞P10′,i.e.L AB represents the linear momentum.In fact,for A= B=0,A=B=1and A=0,B=1the coeﬃcient ofψ20+σ0˙¯σ0in(4.5)is−2ζ(1+ζ¯ζ)−1,2¯ζ(1+ζ¯ζ)−1 and(ζ¯ζ−1)(1+ζ¯ζ)−1,respectively,which are proportional to the independent spatial BMS translations. Therefore,the anti-holomorphic spin-angular momentum can beﬁnite only in the center-of-mass system (i.e.when the spatial components of the Bondi–Sachs energy-momentum are vanishing),and hence I AB in the O(1)part of(4.10)appears to represent only the intrinsic or spin part of the total angular momentum, while rL AB appears to be the orbital part of the angular momentum.To check whether this interpretation is correct we should calculate the quasi-local Pauli–Lubanski spin(2.4)built from the quasi-local anti-holomorphic Dougan–Mason energy-momentum P AB′and the anti-holomorphic spin-angular momentum. However,to compute the spin,we need to know the Dougan–Mason energy-momentum for large spheres with O(r−1)accuracy.Since this has been calculated only in stationary spacetimes[39](where a physical term was apparently overlooked and the gauge ambiguity caused by the spurious solutions was not considered at all),ﬁrst we must calculate this.The r order part of(2.6)is vanishing,because SλA0(0)¯λB′0′(0)d S=2 SλA1(0)¯λB′1′(0)d S=4πσAB′0(seeAppendix A.2),i.e.P AB′rhas aﬁnite r→∞limit at I+.Substituting(3.5)and(3.7)into theﬁnite term of (2.6),using(4.1.a),0′∂′λA0(0)=λA1(0)and its complex conjugate,(4.2.a)and the fact thatψ20+σ0˙¯σ0+0′∂2¯σ0 is real,we obtainS12λA0(1) +λA0(0) 0′∂′¯λB′1′(1)+1Since the last term of the integrand is a total0′∂-derivative:(0′∂2¯σ0)λA0(0)¯λB′0′(0)=0′∂(0′∂¯σ0λA0(0)¯λB′0′(0))−(0′∂¯σ0)λA0(0)0′∂¯λB′0′(0)=0′∂(0′∂¯σ0λA0(0)¯λB′0′(0)−¯σ0λA0(0)¯λB′1′(0)),theﬁnite part of(2.6)is,in fact,the Bondi–Sachs energy-momentum(4.13).The O(r−1)term of(2.6)isSρ′(3)−12 λA0(0)¯λB′0′(2)+λA0(1)¯λB′0′(1)+λA0(2)¯λB′0′(0) −0′∂′λA0(0)¯λB′1′(2)−λA1(2)0′∂¯λB′0′(0)−λA1(1)¯λB′1′(1) d S== S ρ′(3)−12¯λB′0′(2) +¯λB′0′(0) 0′∂λA1(2)+12λA0(1)¯λB′0′(1)−λA1(1)¯λB′1′(1) d S== S − 2Gφ10¯φ1′0+0′∂¯σ00′∂′σ0 λA0(0)¯λB′0′(0)−− 0′∂¯σ0 λA0(0)¯λB′1′(1)− 0′∂′σ0 λA1(1)¯λB′0′(0)+12λA0(1)¯λB′0′(1)− λA1(1)+λA0(0)0′∂¯σ0 ¯λB′1′(1)+¯λB′0′(0)0′∂′σ0 d S.(4.14)Hereﬁrst we used0′∂′λA0(0)=λA1(0),and then(4.3.a),(4.1.b),(3.7)and the fact thatψ20+σ0˙¯σ0+0′∂2¯σ0 is real.However,as we saw above,λA0(1)=C A CλC0(0)=−2C A C0′∂λC1(0),and by means of which(4.2.a) takes the form0′∂(λA1(1)+λA0(0)0′∂¯σ0)=C A C0′∂λC1(0)−(ψ20+σ0˙¯σ0)λA0(0).Since0′∂acting on s=−1r C A CδB′D′+¯C B′D′δA C ∞P CD′−1r M AB′+O(12πSφ10¯φ1′0λA0(0)¯λB′0′(0)d S,(4.16)M AB′:=−12F01′, E01=12(F00′−F11′)and E11=√of ambiguities,and is built only from the gravitational data.Note that our Pauli–Lubanski spin is free of the so-called supertranslation ambiguity,because this is deﬁned in terms of the solutions of an elliptic diﬀerential equation on the cut in question,and not by means of the BMS boost-rotation vectorﬁelds.Thus the present construction is similar in its spirit to that of Penrose[12].The fact that we could derive only a Pauli–Lubanski spin is compatible with the idea of Bergmann and Thomson[21]that the gravitational angular momentum should be analogous to spin(justifying the‘spin-angular momentum’terminology),but raises the question as whether that should be completed by an orbital angular momentum part or not. Another interesting issue is how the Pauli–Lubanski spin changes under(inﬁnitesimal)supertranslations,in particular,under time translations;or whether there exists aﬂux for S AA′through I+or not.However, these questions are beyond the scope of the present paper.5.Stationary spacetimesSuppose that the spacetime is stationary.First we show that all the terms of the integrand of(4.12)involving the asymptotic shear together integrates to zero.Bramson already showed that2¯σ00′∂′σ0+0′∂′(σ0¯σ0)= 0′∂′3(S0′∂2S+12C A BλB0(0)=(GM+0′∂20′∂′2S)λA0(0).Using dim ker′∂(−1,0)=0,this can be solved explicitly.Its solution isλA1(1)=0′∂(0′∂′2S)λA0(0)+(−2GMδA B+C A B)λB1(0).Substituting this into the last term of the integrand,that takes the form−20′∂′σ0 λA1(0) λB1(1)+λB0(0)0′∂¯σ0 +λB1(0) λA1(1)+λA0(0)0′∂¯σ0 ==−2 −2GMδA C+C A C δB D+ −2GMδB D+C B D δA C 0′∂′ σ0λC1(0)λD1(0) .Therefore,the last term of the integrand of(4.12)integrates to zero,too.Byψ20=−Gm the integrand of L AB is the total0′∂′-derivative0′∂′(−GmλA0(0)λB0(0)),and byφ10=14[e2+µ2]λA0(0)λB0(0)).Thus in stationary spacetimes(4.10)reduces toJ AB r =18πSS λA0(0)λB1(0)+λA1(0)λB0(0) d S−3i J m SY1,m S d S and the corresponding realcomponents c i=(c1,c2,c3)by m=1m=−1c m Y1,m=:c1sinθcosφ+c2sinθsinφ+c3cosθ,for the leading terms weﬁnally obtain J ij=εijk j k and J0k=−c k.Thus J AB reproduces the j k’s,and the c k’s can be interpreted as the components of the relativistic center-of-mass.In particular,for the Kerr–Newman solution on the shear-free cuts J12=−ing the expressions of Appendix A.2for the L2scalar product of the spinorcomponentsλA A2(e2+µ2)σAB′and M AB′=−2GM2σAB′.Hence in stationary spacetimes the Pauli–Lubanski spin reduces to that of Bramson.As he showed[23],this is invariant with respect to supertranslations.。

Asymptotic expansions for ratios of products of gamma functions

a r X i v :m a t h /0206200v 2 [m a t h .C A ] 27 M a y 2003Asymptotic expansions for ratios of productsof gamma functionsWolfgang B¨u hringPhysikalisches Institut,Universit¨a t Heidelberg,Philosophenweg 1269120Heidelberg,Germanybuehring@physi.uni-heidelberg.deAbstractAn asymptotic expansion for a ratio of products of gamma func-tions is derived.2000Mathematics Subject Classiﬁcation:Primary 33B15;Secondary 33C20Keywords and phrases:Gamma function,generalized hypergeometric functions1IntroductionAn asymptotic expansion for a ratio of products of gamma functions has recently been found [2],which,withs 1=b 1−a 1−a 2,(1)may be writtenΓ(a 1+n )Γ(a 2+n )(1)m (1+s 1−n )m +O (n −M −1)(2)1as n→∞.Here use is made of the Pochhammer symbol(x)n=x(x+1)···(x+n−1)=Γ(x+n)/Γ(x).The special case when b1=1of this formula(2)had been stated earlierby Dingle[3],and there wereproofs by Paris[8]and Olver[6][7].The proof of(2)is based on the formula for the analytic continuation near unit argument of the Gaussian hypergeometric function.For the more general hypergeometric functionsp+1F p a1,a2,...,a p+1b1,...,b p z=∞n=0(a1)n(a2)n···(a p+1)nΓ(b1)···Γ(b p)p+1F p a1,a2,...,a p+1b1,...,b p z(4)=∞m=0g m(0)(1−z)m+(1−z)s p∞ m=0g m(s p)(1−z)m,wheres p=b1+···+b p−a1−a2−···−a p+1(5) and the coeﬃcients g m are known.While the g m(0)are not needed for the present purpose,the g m(s p)are[1]g m(s p)=(−1)m(a1+s p)m(a2+s p)mΓ(−s p−m)(a1+s p)k(a2+s p)kA(p)k,2where the coeﬃcients A(p)k will be shown below.The left-hand side L of(4)isL=∞n=0Γ(a1+n)Γ(a2+n)···Γ(a p+1+n)Γ(1+n)z n.(9)Interchanging the order of summation(and making use of the reﬂection for-mula of the gamma function)we may getR=∞n=0Γ(−s p+n)Γ(−s p−m)(1+s p−n)m z n.(10)Comparison of the coeﬃcients of the two power series for R and L,which asymptotically,as n→∞,should agree,then leads toΓ(a1+n)Γ(a2+n)···Γ(a p+1+n)Γ(−s p−m)(1+s p−n)m.Inserting g m from(6)and keeping theﬁrst M+1terms of the asymptotic series,we getTheorem1Γ(a1+n)Γ(a2+n)···Γ(a p+1+n)(1)m(1+s p−n)mmk=0(−m)kThe simple formula(2)above corresponding to p=1can be recovered from this theorem if we deﬁne A(1)0=1,A(1)k=0for k>0,so that then the sum over k is equal to1and disappears.The coeﬃcients for larger p can be found in[1],but a few of them are here displayed again for convenience:A(2)k=(b2−a3)k(b1−a3)k(k−k2)!k2!,(14)A(4)k=kk2=0(b4+b3+b2−a5−a4−a3+k2)k−k2(b1−a3)k−k2(k2−k3)!×(b4−a5)k3(b3−a5)k3k!(16)×3F2 b3−a4,b2−a4,−kb3+b2−a4−a3,1+a3−b1−k 1orA(3)k=(b1+b3−a3−a4)k(b2+b3−a3−a4)kΓ(b1+n)Γ(b2+n)Γ(−s2+n)=1(18)+Mm=1(a1+s2)m(a2+s2)m3Additional commentsThe derivation of the theorem is based on the continuation formula(4)which holds,as it stands,only if s p is not equal to an integer.Nevertheless,the the-orem is valid without such a restriction.This can be veriﬁed if the derivation is repeated starting from any of the continuation formulas for the exceptional cases[1].Instead of or in addition to the binomial theorem,the expansion(1−z)m ln(1−z)=∞n=1c n z n,(19)is then needed for integer m≥0,wherec n=−1Γ(n)(20)for n>m,while the coeﬃcients are not needed here for n≤m.The theorem has been proved here for any suﬃciently large positive in-teger n only.On the basis of the discussion in[2],it can be suspected that the theorem may be theoretically valid(although less useful)in the larger domain of the complex n-half-plane Re(s p+a1+a2−1+n)≥0.Expansions for ratios of even more general products of gamma functions are treated in a recent monograph by Paris and Kaminski[9]. References[1]W.B¨u hring,Generalized hypergeometric functions at unit argument,Proc.Amer Math.Soc.114(1992),145–153.[2]W.B¨u hring,An asymptotic expansion for a ratio of products ofgamma functions,Internat.J.Math.&Math.Sci.24(2000),504–510.[3]R.B.Dingle,Asymptotic Expansions:Their Derivation and Inter-pretation(Academic Press,London,1973).[4]P.Flajolet and A.Odlyzko,Singularity analysis of generating func-tions,SIAM J.Discrete Math.3(1990),216–240.[5]F.W.J.Olver,Asymptotics and Special Functions(Academic Press,New York,1974).5[6]F.W.J.Olver,Asymptotic expansions of the coeﬃcients in asymp-totic series solutions of linear diﬀerential equations,Methods and Applications of Analysis1(1994),1–13.[7]F.W.J.Olver,On an asymptotic expansion of a ratio of gammafunctions,Proc.Royal Irish Acad.A95(1995),5–9.[8]R.B.Paris,Smoothing of the Stokes phenomenon using Mellin-Barnes integrals,put.Appl.Math.41(1992),117–133. [9]R.B.Paris and D.Kaminski,Asymptotics and Mellin-Barnes inte-grals,Cambridge University Press,Cambridge,2001.[10]R.Sch¨a fke and D.Schmidt,The connection problem for general lin-ear ordinary diﬀerential equations at two regular singular points with applications in the theory of special functions,SIAM J.Math.Anal.11(1980),848–862.6。

乳液聚合胶束成核机理谁提出来的对应的英文文章

乳液聚合胶束成核机理谁提出来的对应的英文文章乳液聚合胶束成核机理是由法国物理学家Jean-Pierre Chapel提出的。

该理论在1971年由他在《Journal of Colloid and Interface Science》发表的一篇名为"Polymerization of Micelles: A Phenomenological Approach"的英文文章中详细阐述。

后附译文Introduction:Emulsion polymerization is a widely used technique for the synthesis of various polymers. The process involves the formation of polymer particles in a water-insoluble monomer phase dispersed in water through the use of surfactants and emulsifiers. The understanding of the nucleation mechanism in this process is crucial for optimizing the synthesis and controlling the particle size and morphology. In this regard, the groundbreaking work of Jean-Pierre Chapel on the mechanism of micelle nucleation in emulsion polymerization provides valuable insights and has been of significant interest to researchers.Brief Background:Emulsion polymerization involves the formation of micelles, which are colloidal aggregates of surfactant molecules, to stabilize the monomer droplets in water. These micelles act as the nucleation sites for the polymerization reaction. Jean-Pierre Chapel proposed a phenomenological approach to explain the micelle nucleation process in emulsion polymerization. His work focused on understandingthe role of surfactants and their interactions with the monomer molecules in the nucleation process.Chapel's Phenomenological Approach:Chapel's approach involved the use of classical thermodynamics to model the micelle nucleation mechanism in emulsion polymerization. He considered the system as a two-phase mixture of monomer droplets dispersed in water and the impact of surfactant molecules on the nucleation process. Chapel formulated his theory based on well-established thermodynamic principles and made a few key assumptions.Assumptions:1. The surfactant molecules are assumed to spontaneously adsorb at the monomer-water interface due to the hydrophobicity of the monomers.2. The adsorption of surfactant at the monomer-water interface leads to the formation of a monolayer around the monomer droplet, stabilizing it against coalescence.3. Polymerization occurs within the surfactant-stabilized monomer droplets.Theoretical Explanation:Chapel's phenomenological approach involved the use of classical nucleation theory and the Gibbs free energy change associated with micelle formation. He derived equations that describe the change in free energy due to the adsorption of surfactant molecules at the monomer-water interface, the deformation of the surfactant monolayer, and the formation of micelles. Chapel recognized that the monomer-water interfaceequilibrium must be considered in the calculations. His model allowed for the prediction of the critical micelle concentration (CMC) and the rate of polymerization based on the thermodynamic parameters of the system.Significance of Chapel's Work:Chapel's model provided a deeper understanding of the nucleation process in emulsion polymerization. His approach allowed for the prediction and control of the CMC, which is a critical parameter in determining the stability of the emulsion and the particle size distribution. Chapel's work also highlighted the importance of surfactant properties, such as hydrophobicity and molecule structure, in the nucleation and stabilization processes. This knowledge has been invaluable for the design and synthesis of emulsion polymerization systems with desired properties.Further Research and Applications:Since Chapel's seminal work, researchers have built upon his model and expanded the understanding of emulsion polymerization mechanisms. The development of more efficient and versatile surfactants, advancements in experimental techniques, and computational modeling have further enhanced the understanding of the nucleation process. This knowledge has led to the development of new emulsion polymerization techniques and the synthesis of polymers with tailored properties for a wide range of applications, including coatings, adhesives, and biomaterials.Conclusion:Jean-Pierre Chapel's phenomenological approach to understanding the micelle nucleation mechanism in emulsion polymerization has provided valuable insights into the roleof surfactants in this process. His work has laid the foundation for further research in the field and has contributed significantly to the design and synthesis of polymer particles with controlled properties. The understanding of the nucleation mechanism is crucial for optimizing emulsion polymerization processes and enables the production of polymers for diverse applications.乳液聚合胶束成核机理是由法国物理学家Jean-Pierre Chapel提出的.该理论在1971年由他在《胶体和界面科学杂志》发表的一篇名为“胶束聚合：现象学方法”的英文文章中详细阐述。

非等位基因

非等位基因概述非等位基因是指同一基因座上的不同等位基因。

等位基因是指在某个给定的基因座上，可以存在多种不同的变体。

每个个体继承了一对等位基因，一对等位基因可能会导致不同的表型表达。

非等位基因的存在使得遗传学研究更加复杂，因为不同的等位基因会对个体的表型产生不同的影响。

背景在生物学中，基因座是指染色体上一个特定的位置，该位置上的基因决定了某个特征的表达方式。

每个基因座上可以有多种不同的等位基因。

等位基因是指在某个特定基因座上的不同基因变体。

每个个体都会继承一对等位基因，通过这对等位基因的不同组合，决定了个体的表型。

然而，并非所有基因座上的等位基因都具有相同的表现型。

非等位基因的影响非等位基因的存在导致不同等位基因会对个体表型产生不同的影响。

有些非等位基因会表现出显性效应，也就是说，当个体继承了一个突变的等位基因时，即使同时继承了一个正常的等位基因，但显性效应会使得突变的等位基因的表型表达得到体现。

相反，有些非等位基因会表现出隐性效应，当个体继承了两个突变的等位基因时，才会表现出突变的表型。

除了显性和隐性效应之外，非等位基因还可能发生两种其他类型的表型效应。

一种是共显效应，当个体继承了两个不同的突变等位基因时，在表型表达上会表现出一种新的特征，这个特征并不是单个突变等位基因所能导致的。

另一种是部分显性效应，当个体继承了两个不同的突变等位基因时，表型表达将介于两个单独突变等位基因的表型之间。

重组和非等位基因重组是指两个不同的染色体交换部分基因序列的过程。

在重组的过程中，非等位基因可能会发生改变，导致新的等位基因组合形成。

这一过程使得非等位基因的表型效应更加复杂，因为新的等位基因可能将不同基因座的效应组合起来。

非等位基因的重要性非等位基因对生物的适应性和多样性起着重要作用。

通过对等位基因的各种组合的研究，人们可以更好地理解基因与表型之间的关系，并揭示遗传变异对物种适应环境的重要性。

总结非等位基因是指同一基因座上的不同等位基因。

不对称自由基反应英文

不对称自由基反应英文Asymmetric Radical Reactions: An Insight into Their Mechanism and Applications.Introduction.Asymmetric radical reactions have emerged as a powerful tool in organic synthesis, enabling the synthesis of chiral compounds with high enantiomeric purity. These reactions differ significantly from their symmetric counterparts, as they involve the generation and utilization of chiral radicals. These chiral radicals can undergo a range of reactions, including substitution, addition, and cyclization, leading to the formation of enantiomerically enriched products.Mechanism of Asymmetric Radical Reactions.The mechanism of asymmetric radical reactions typically involves three key steps: radical generation, chiralitytransfer, and radical termination.Radical Generation.The first step involves the generation of a radical species. This can be achieved through various methods, such as photolysis, thermal decomposition, or redox reactions. The generated radical can be chiral or achiral, depending on the starting materials and the conditions used.Chirality Transfer.The second step involves the transfer of chirality from a chiral auxiliary or catalyst to the radical species. This chirality transfer can occur through covalent or non-covalent interactions between the catalyst/auxiliary and the radical. The nature of these interactions determines the stereoselectivity of the reaction.Radical Termination.The final step involves the termination of the radicalspecies, leading to the formation of the desired product. This termination can occur through various mechanisms, such as coupling with another radical species, hydrogen atom abstraction, or disproportionation.Applications of Asymmetric Radical Reactions.Asymmetric radical reactions have found widespread applications in various fields of organic synthesis, including the synthesis of natural products, pharmaceuticals, and functional materials.Synthesis of Natural Products.Natural products often possess complex chiral structures, making their synthesis challenging. Asymmetric radical reactions have proven to be effective tools for the synthesis of such chiral natural products. For example, the use of chiral radicals generated from appropriate precursors has enabled the enantioselective synthesis of alkaloids, terpenes, and amino acids.Pharmaceutical Applications.The enantiomers of chiral drugs often differ significantly in their biological activities, making it crucial to control their enantiomeric purity. Asymmetric radical reactions can be used to synthesize enantiomerically enriched chiral drugs with high selectivity. This approach has been successfully applied to the synthesis of various drugs, including anti-inflammatory agents, anticancer agents, and antiviral agents.Functional Materials.Chiral materials possess unique physical and chemical properties that make them useful in various applications, such as displays, sensors, and catalysts. Asymmetricradical reactions can be used to synthesize chiral building blocks for the preparation of such materials. For instance, chiral polymers can be synthesized by utilizing asymmetric radical polymerization reactions, leading to the formation of materials with controlled chirality and tailored properties.Conclusion.Asymmetric radical reactions have emerged as powerful tools for the synthesis of enantiomerically enriched chiral compounds. Their unique mechanism, involving chirality transfer from a chiral catalyst/auxiliary to the radical species, enables high selectivity and enantiopurity in the product. The widespread applications of asymmetric radical reactions in organic synthesis, particularly in the synthesis of natural products, pharmaceuticals, and functional materials, highlight their importance in modern chemistry.Future Perspectives.Despite the significant progress made in the field of asymmetric radical reactions, there are still numerous challenges and opportunities for further exploration.Improving Selectivity and Efficiency.One of the key challenges in asymmetric radical reactions is achieving high selectivity and efficiency. While significant progress has been made in this area, there is still room for improvement. Future research could focus on developing new chiral catalysts/auxiliaries that can promote asymmetric radical reactions with higher selectivity and efficiency.Expanding the Scope of Reactions.Currently, the scope of asymmetric radical reactions is limited by the availability of suitable precursors and the reactivity of the generated radicals. Future research could aim to expand the scope of these reactions by developing new methods for generating radicals with desired functionalities and reactivities.Applications in Sustainable Chemistry.In the context of sustainable chemistry, asymmetric radical reactions offer an attractive alternative to traditional synthetic methods. By utilizing renewableresources and mild reaction conditions, asymmetric radical reactions could contribute to the development of more sustainable synthetic routes for the preparation of chiral compounds.Integration with Other Techniques.The integration of asymmetric radical reactions with other techniques, such as photocatalysis, electrochemistry, and microfluidics, could lead to the development of new and innovative synthetic methods. By combining the advantages of these techniques, it may be possible to achieve even higher selectivity, efficiency, and scalability in asymmetric radical reactions.In conclusion, asymmetric radical reactions have emerged as powerful tools for the synthesis of enantiomerically enriched chiral compounds. While significant progress has been made in this area, there are still numerous opportunities for further exploration and development. Future research in this field could lead tothe discovery of new and innovative synthetic methods with improved selectivity, efficiency, and sustainability.。

开启片剂完整性的窗户(中英文对照)

开启片剂完整性的窗户日本东芝公司，剑桥大学摘要：由日本东芝公司和剑桥大学合作成立的公司向《医药技术》解释了FDA支持的技术如何在不损坏片剂的情况下测定其完整性。

太赫脉冲成像的一个应用是检查肠溶制剂的完整性，以确保它们在到达肠溶之前不会溶解。

关键词：片剂完整性，太赫脉冲成像。

能够检测片剂的结构完整性和化学成分而无需将它们打碎的一种技术，已经通过了概念验证阶段，正在进行法规申请。

由英国私募Teraview公司研发并且以太赫光（介于无线电波和光波之间）为基础。

该成像技术为配方研发和质量控制中的湿溶出试验提供了一个更好的选择。

该技术还可以缩短新产品的研发时间，并且根据厂商的情况，随时间推移甚至可能发展成为一个用于制药生产线的实时片剂检测系统。

TPI技术通过发射太赫射线绘制出片剂和涂层厚度的三维差异图谱，在有结构或化学变化时太赫射线被反射回。

反射脉冲的时间延迟累加成该片剂的三维图像。

该系统使用太赫发射极，采用一个机器臂捡起片剂并且使其通过太赫光束，用一个扫描仪收集反射光并且建成三维图像(见图)。

技术研发太赫技术发源于二十世纪九十年代中期13本东芝公司位于英国的东芝欧洲研究中心，该中心与剑桥大学的物理学系有着密切的联系。

日本东芝公司当时正在研究新一代的半导体，研究的副产品是发现了这些半导体实际上是太赫光非常好的发射源和检测器。

二十世纪九十年代后期，日本东芝公司授权研究小组寻求该技术可能的应用，包括成像和化学传感光谱学，并与葛兰素史克和辉瑞以及其它公司建立了关系，以探讨其在制药业的应用。

虽然早期的结果表明该技术有前景，但日本东芝公司却不愿深入研究下去，原因是此应用与日本东芝公司在消费电子行业的任何业务兴趣都没有交叉。

这一决定的结果是研究中心的首席执行官DonArnone和剑桥桥大学物理学系的教授Michael Pepper先生于2001年成立了Teraview公司一作为研究中心的子公司。

TPI imaga 2000是第一个商品化太赫成像系统，该系统经优化用于成品片剂及其核心完整性和性能的无破坏检测。

以英文命名的物理学现象

以英文命名的物理学现象
以下是一些以英文命名的物理学现象：
1. Doppler Effect（多普勒效应）：描述当波源相对于观察者运动时，观察到的波的频率和波长发生变化的现象。

2. Photoelectric Effect（光电效应）：指的是当光照射到金属表面时，光子的能量足够大时，可以将金属中的电子从原子中解离出来形成电流的现象。

3. Quantum Tunneling（量子隧道效应）：是指在经典物理学下不可能发生的情况下，由于量子力学的特性，粒子可以穿越能量势垒的现象。

4. Superconductivity（超导现象）：指的是在极低温度下，某些特定材料的电阻消失，可以使电流在其中无阻碍地流动的现象。

5. Bose-Einstein Condensation（玻色-爱因斯坦凝聚）：是指在极低温下，玻色子（一种基本粒子）的量子态出现互不冲突的大量粒子处于相同量子态的集体行为。

请注意，以上内容仅为物理学现象的一部分，如您对其他领域的命名有需要，请提供更具体的问题。

专八英语阅读

英语专业八级考试TEM-8阅读理解练习册（1）(英语专业2012级)UNIT 1Text AEvery minute of every day, what ecologist生态学家James Carlton calls a global ―conveyor belt‖, redistributes ocean organisms生物.It’s planetwide biological disruption生物的破坏that scientists have barely begun to understand.Dr. Carlton —an oceanographer at Williams College in Williamstown,Mass.—explains that, at any given moment, ―There are several thousand marine species traveling… in the ballast water of ships.‖ These creatures move from coastal waters where they fit into the local web of life to places where some of them could tear that web apart. This is the larger dimension of the infamous无耻的，邪恶的invasion of fish-destroying, pipe-clogging zebra mussels有斑马纹的贻贝.Such voracious贪婪的invaders at least make their presence known. What concerns Carlton and his fellow marine ecologists is the lack of knowledge about the hundreds of alien invaders that quietly enter coastal waters around the world every day. Many of them probably just die out. Some benignly亲切地，仁慈地—or even beneficially — join the local scene. But some will make trouble.In one sense, this is an old story. Organisms have ridden ships for centuries. They have clung to hulls and come along with cargo. What’s new is the scale and speed of the migrations made possible by the massive volume of ship-ballast water压载水— taken in to provide ship stability—continuously moving around the world…Ships load up with ballast water and its inhabitants in coastal waters of one port and dump the ballast in another port that may be thousands of kilometers away. A single load can run to hundreds of gallons. Some larger ships take on as much as 40 million gallons. The creatures that come along tend to be in their larva free-floating stage. When discharged排出in alien waters they can mature into crabs, jellyfish水母, slugs鼻涕虫，蛞蝓, and many other forms.Since the problem involves coastal species, simply banning ballast dumps in coastal waters would, in theory, solve it. Coastal organisms in ballast water that is flushed into midocean would not survive. Such a ban has worked for North American Inland Waterway. But it would be hard to enforce it worldwide. Heating ballast water or straining it should also halt the species spread. But before any such worldwide regulations were imposed, scientists would need a clearer view of what is going on.The continuous shuffling洗牌of marine organisms has changed the biology of the sea on a global scale. It can have devastating effects as in the case of the American comb jellyfish that recently invaded the Black Sea. It has destroyed that sea’s anchovy鳀鱼fishery by eating anchovy eggs. It may soon spread to western and northern European waters.The maritime nations that created the biological ―conveyor belt‖ should support a coordinated international effort to find out what is going on and what should be done about it. (456 words)1.According to Dr. Carlton, ocean organism‟s are_______.A.being moved to new environmentsB.destroying the planetC.succumbing to the zebra musselD.developing alien characteristics2.Oceanographers海洋学家are concerned because_________.A.their knowledge of this phenomenon is limitedB.they believe the oceans are dyingC.they fear an invasion from outer-spaceD.they have identified thousands of alien webs3.According to marine ecologists, transplanted marinespecies____________.A.may upset the ecosystems of coastal watersB.are all compatible with one anotherC.can only survive in their home watersD.sometimes disrupt shipping lanes4.The identified cause of the problem is_______.A.the rapidity with which larvae matureB. a common practice of the shipping industryC. a centuries old speciesD.the world wide movement of ocean currents5.The article suggests that a solution to the problem__________.A.is unlikely to be identifiedB.must precede further researchC.is hypothetically假设地，假想地easyD.will limit global shippingText BNew …Endangered‟ List Targets Many US RiversIt is hard to think of a major natural resource or pollution issue in North America today that does not affect rivers.Farm chemical runoff残渣, industrial waste, urban storm sewers, sewage treatment, mining, logging, grazing放牧,military bases, residential and business development, hydropower水力发电,loss of wetlands. The list goes on.Legislation like the Clean Water Act and Wild and Scenic Rivers Act have provided some protection, but threats continue.The Environmental Protection Agency (EPA) reported yesterday that an assessment of 642,000 miles of rivers and streams showed 34 percent in less than good condition. In a major study of the Clean Water Act, the Natural Resources Defense Council last fall reported that poison runoff impairs损害more than 125,000 miles of rivers.More recently, the NRDC and Izaak Walton League warned that pollution and loss of wetlands—made worse by last year’s flooding—is degrading恶化the Mississippi River ecosystem.On Tuesday, the conservation group保护组织American Rivers issued its annual list of 10 ―endangered‖ and 20 ―threatened‖ rivers in 32 states, the District of Colombia, and Canada.At the top of the list is the Clarks Fork of the Yellowstone River, whereCanadian mining firms plan to build a 74-acre英亩reservoir水库，蓄水池as part of a gold mine less than three miles from Yellowstone National Park. The reservoir would hold the runoff from the sulfuric acid 硫酸used to extract gold from crushed rock.―In the event this tailings pond failed, the impact to th e greater Yellowstone ecosystem would be cataclysmic大变动的，灾难性的and the damage irreversible不可逆转的.‖ Sen. Max Baucus of Montana, chairman of the Environment and Public Works Committee, wrote to Noranda Minerals Inc., an owner of the ― New World Mine‖.Last fall, an EPA official expressed concern about the mine and its potential impact, especially the plastic-lined storage reservoir. ― I am unaware of any studies evaluating how a tailings pond尾矿池，残渣池could be maintained to ensure its structural integrity forev er,‖ said Stephen Hoffman, chief of the EPA’s Mining Waste Section. ―It is my opinion that underwater disposal of tailings at New World may present a potentially significant threat to human health and the environment.‖The results of an environmental-impact statement, now being drafted by the Forest Service and Montana Department of State Lands, could determine the mine’s future…In its recent proposal to reauthorize the Clean Water Act, the Clinton administration noted ―dramatically improved water quality since 1972,‖ when the act was passed. But it also reported that 30 percent of riverscontinue to be degraded, mainly by silt泥沙and nutrients from farm and urban runoff, combined sewer overflows, and municipal sewage城市污水. Bottom sediments沉积物are contaminated污染in more than 1,000 waterways, the administration reported in releasing its proposal in January. Between 60 and 80 percent of riparian corridors (riverbank lands) have been degraded.As with endangered species and their habitats in forests and deserts, the complexity of ecosystems is seen in rivers and the effects of development----beyond the obvious threats of industrial pollution, municipal waste, and in-stream diversions改道to slake消除the thirst of new communities in dry regions like the Southwes t…While there are many political hurdles障碍ahead, reauthorization of the Clean Water Act this year holds promise for US rivers. Rep. Norm Mineta of California, who chairs the House Committee overseeing the bill, calls it ―probably the most important env ironmental legislation this Congress will enact.‖ (553 words)6.According to the passage, the Clean Water Act______.A.has been ineffectiveB.will definitely be renewedC.has never been evaluatedD.was enacted some 30 years ago7.“Endangered” rivers are _________.A.catalogued annuallyB.less polluted than ―threatened rivers‖C.caused by floodingD.adjacent to large cities8.The “cataclysmic” event referred to in paragraph eight would be__________.A. fortuitous偶然的，意外的B. adventitious外加的，偶然的C. catastrophicD. precarious不稳定的，危险的9. The owners of the New World Mine appear to be______.A. ecologically aware of the impact of miningB. determined to construct a safe tailings pondC. indifferent to the concerns voiced by the EPAD. willing to relocate operations10. The passage conveys the impression that_______.A. Canadians are disinterested in natural resourcesB. private and public environmental groups aboundC. river banks are erodingD. the majority of US rivers are in poor conditionText CA classic series of experiments to determine the effects ofoverpopulation on communities of rats was reported in February of 1962 in an article in Scientific American. The experiments were conducted by a psychologist, John B. Calhoun and his associates. In each of these experiments, an equal number of male and female adult rats were placed in an enclosure and given an adequate supply of food, water, and other necessities. The rat populations were allowed to increase. Calhoun knew from experience approximately how many rats could live in the enclosures without experiencing stress due to overcrowding. He allowed the population to increase to approximately twice this number. Then he stabilized the population by removing offspring that were not dependent on their mothers. He and his associates then carefully observed and recorded behavior in these overpopulated communities. At the end of their experiments, Calhoun and his associates were able to conclude that overcrowding causes a breakdown in the normal social relationships among rats, a kind of social disease. The rats in the experiments did not follow the same patterns of behavior as rats would in a community without overcrowding.The females in the rat population were the most seriously affected by the high population density: They showed deviant异常的maternal behavior; they did not behave as mother rats normally do. In fact, many of the pups幼兽，幼崽, as rat babies are called, died as a result of poor maternal care. For example, mothers sometimes abandoned their pups,and, without their mothers' care, the pups died. Under normal conditions, a mother rat would not leave her pups alone to die. However, the experiments verified that in overpopulated communities, mother rats do not behave normally. Their behavior may be considered pathologically 病理上，病理学地diseased.The dominant males in the rat population were the least affected by overpopulation. Each of these strong males claimed an area of the enclosure as his own. Therefore, these individuals did not experience the overcrowding in the same way as the other rats did. The fact that the dominant males had adequate space in which to live may explain why they were not as seriously affected by overpopulation as the other rats. However, dominant males did behave pathologically at times. Their antisocial behavior consisted of attacks on weaker male,female, and immature rats. This deviant behavior showed that even though the dominant males had enough living space, they too were affected by the general overcrowding in the enclosure.Non-dominant males in the experimental rat communities also exhibited deviant social behavior. Some withdrew completely; they moved very little and ate and drank at times when the other rats were sleeping in order to avoid contact with them. Other non-dominant males were hyperactive; they were much more active than is normal, chasing other rats and fighting each other. This segment of the rat population, likeall the other parts, was affected by the overpopulation.The behavior of the non-dominant males and of the other components of the rat population has parallels in human behavior. People in densely populated areas exhibit deviant behavior similar to that of the rats in Calhoun's experiments. In large urban areas such as New York City, London, Mexican City, and Cairo, there are abandoned children. There are cruel, powerful individuals, both men and women. There are also people who withdraw and people who become hyperactive. The quantity of other forms of social pathology such as murder, rape, and robbery also frequently occur in densely populated human communities. Is the principal cause of these disorders overpopulation? Calhoun’s experiments suggest that it might be. In any case, social scientists and city planners have been influenced by the results of this series of experiments.11. Paragraph l is organized according to__________.A. reasonsB. descriptionC. examplesD. definition12．Calhoun stabilized the rat population_________.A. when it was double the number that could live in the enclosure without stressB. by removing young ratsC. at a constant number of adult rats in the enclosureD. all of the above are correct13.W hich of the following inferences CANNOT be made from theinformation inPara. 1?A. Calhoun's experiment is still considered important today.B. Overpopulation causes pathological behavior in rat populations.C. Stress does not occur in rat communities unless there is overcrowding.D. Calhoun had experimented with rats before.14. Which of the following behavior didn‟t happen in this experiment?A. All the male rats exhibited pathological behavior.B. Mother rats abandoned their pups.C. Female rats showed deviant maternal behavior.D. Mother rats left their rat babies alone.15. The main idea of the paragraph three is that __________.A. dominant males had adequate living spaceB. dominant males were not as seriously affected by overcrowding as the otherratsC. dominant males attacked weaker ratsD. the strongest males are always able to adapt to bad conditionsText DThe first mention of slavery in the statutes法令，法规of the English colonies of North America does not occur until after 1660—some forty years after the importation of the first Black people. Lest we think that existed in fact before it did in law, Oscar and Mary Handlin assure us, that the status of B lack people down to the 1660’s was that of servants. A critique批判of the Handlins’ interpretation of why legal slavery did not appear until the 1660’s suggests that assumptions about the relation between slavery and racial prejudice should be reexamined, and that explanation for the different treatment of Black slaves in North and South America should be expanded.The Handlins explain the appearance of legal slavery by arguing that, during the 1660’s, the position of white servants was improving relative to that of black servants. Thus, the Handlins contend, Black and White servants, heretofore treated alike, each attained a different status. There are, however, important objections to this argument. First, the Handlins cannot adequately demonstrate that t he White servant’s position was improving, during and after the 1660’s; several acts of the Maryland and Virginia legislatures indicate otherwise. Another flaw in the Handlins’ interpretation is their assumption that prior to the establishment of legal slavery there was no discrimination against Black people. It is true that before the 1660’s Black people were rarely called slaves. But this shouldnot overshadow evidence from the 1630’s on that points to racial discrimination without using the term slavery. Such discrimination sometimes stopped short of lifetime servitude or inherited status—the two attributes of true slavery—yet in other cases it included both. The Handlins’ argument excludes the real possibility that Black people in the English colonies were never treated as the equals of White people.The possibility has important ramifications后果，影响.If from the outset Black people were discriminated against, then legal slavery should be viewed as a reflection and an extension of racial prejudice rather than, as many historians including the Handlins have argued, the cause of prejudice. In addition, the existence of discrimination before the advent of legal slavery offers a further explanation for the harsher treatment of Black slaves in North than in South America. Freyre and Tannenbaum have rightly argued that the lack of certain traditions in North America—such as a Roman conception of slavery and a Roman Catholic emphasis on equality— explains why the treatment of Black slaves was more severe there than in the Spanish and Portuguese colonies of South America. But this cannot be the whole explanation since it is merely negative, based only on a lack of something. A more compelling令人信服的explanation is that the early and sometimes extreme racial discrimination in the English colonies helped determine the particular nature of the slavery that followed. (462 words)16. Which of the following is the most logical inference to be drawn from the passage about the effects of “several acts of the Maryland and Virginia legislatures” (Para.2) passed during and after the 1660‟s?A. The acts negatively affected the pre-1660’s position of Black as wellas of White servants.B. The acts had the effect of impairing rather than improving theposition of White servants relative to what it had been before the 1660’s.C. The acts had a different effect on the position of white servants thandid many of the acts passed during this time by the legislatures of other colonies.D. The acts, at the very least, caused the position of White servants toremain no better than it had been before the 1660’s.17. With which of the following statements regarding the status ofBlack people in the English colonies of North America before the 1660‟s would the author be LEAST likely to agree?A. Although black people were not legally considered to be slaves,they were often called slaves.B. Although subject to some discrimination, black people had a higherlegal status than they did after the 1660’s.C. Although sometimes subject to lifetime servitude, black peoplewere not legally considered to be slaves.D. Although often not treated the same as White people, black people,like many white people, possessed the legal status of servants.18. According to the passage, the Handlins have argued which of thefollowing about the relationship between racial prejudice and the institution of legal slavery in the English colonies of North America?A. Racial prejudice and the institution of slavery arose simultaneously.B. Racial prejudice most often the form of the imposition of inheritedstatus, one of the attributes of slavery.C. The source of racial prejudice was the institution of slavery.D. Because of the influence of the Roman Catholic Church, racialprejudice sometimes did not result in slavery.19. The passage suggests that the existence of a Roman conception ofslavery in Spanish and Portuguese colonies had the effect of _________.A. extending rather than causing racial prejudice in these coloniesB. hastening the legalization of slavery in these colonies.C. mitigating some of the conditions of slavery for black people in these coloniesD. delaying the introduction of slavery into the English colonies20. The author considers the explanation put forward by Freyre andTannenbaum for the treatment accorded B lack slaves in the English colonies of North America to be _____________.A. ambitious but misguidedB. valid有根据的but limitedC. popular but suspectD. anachronistic过时的，时代错误的and controversialUNIT 2Text AThe sea lay like an unbroken mirror all around the pine-girt, lonely shores of Orr’s Island. Tall, kingly spruce s wore their regal王室的crowns of cones high in air, sparkling with diamonds of clear exuded gum流出的树胶; vast old hemlocks铁杉of primeval原始的growth stood darkling in their forest shadows, their branches hung with long hoary moss久远的青苔;while feathery larches羽毛般的落叶松,turned to brilliant gold by autumn frosts, lighted up the darker shadows of the evergreens. It was one of those hazy朦胧的, calm, dissolving days of Indian summer, when everything is so quiet that the fainest kiss of the wave on the beach can be heard, and white clouds seem to faint into the blue of the sky, and soft swathing一长条bands of violet vapor make all earth look dreamy, and give to the sharp, clear-cut outlines of the northern landscape all those mysteries of light and shade which impart such tenderness to Italian scenery.The funeral was over,--- the tread鞋底的花纹/ 踏of many feet, bearing the heavy burden of two broken lives, had been to the lonely graveyard, and had come back again,--- each footstep lighter and more unconstrained不受拘束的as each one went his way from the great old tragedy of Death to the common cheerful of Life.The solemn black clock stood swaying with its eternal ―tick-tock, tick-tock,‖ in the kitchen of the brown house on Orr’s Island. There was there that sense of a stillness that can be felt,---such as settles down on a dwelling住处when any of its inmates have passed through its doors for the last time, to go whence they shall not return. The best room was shut up and darkened, with only so much light as could fall through a little heart-shaped hole in the window-shutter,---for except on solemn visits, or prayer-meetings or weddings, or funerals, that room formed no part of the daily family scenery.The kitchen was clean and ample, hearth灶台, and oven on one side, and rows of old-fashioned splint-bottomed chairs against the wall. A table scoured to snowy whiteness, and a little work-stand whereon lay the Bible, the Missionary Herald, and the Weekly Christian Mirror, before named, formed the principal furniture. One feature, however, must not be forgotten, ---a great sea-chest水手用的储物箱,which had been the companion of Zephaniah through all the countries of the earth. Old, and battered破旧的，磨损的, and unsightly难看的it looked, yet report said that there was good store within which men for the most part respect more than anything else; and, indeed it proved often when a deed of grace was to be done--- when a woman was suddenly made a widow in a coast gale大风，狂风, or a fishing-smack小渔船was run down in the fogs off the banks, leaving in some neighboring cottage a family of orphans,---in all such cases, the opening of this sea-chest was an event of good omen 预兆to the bereaved丧亲者;for Zephaniah had a large heart and a large hand, and was apt有…的倾向to take it out full of silver dollars when once it went in. So the ark of the covenant约柜could not have been looked on with more reverence崇敬than the neighbours usually showed to Captain Pennel’s sea-chest.1. The author describes Orr‟s Island in a(n)______way.A.emotionally appealing, imaginativeB.rational, logically preciseC.factually detailed, objectiveD.vague, uncertain2.According to the passage, the “best room”_____.A.has its many windows boarded upB.has had the furniture removedC.is used only on formal and ceremonious occasionsD.is the busiest room in the house3.From the description of the kitchen we can infer that thehouse belongs to people who_____.A.never have guestsB.like modern appliancesC.are probably religiousD.dislike housework4.The passage implies that_______.A.few people attended the funeralB.fishing is a secure vocationC.the island is densely populatedD.the house belonged to the deceased5.From the description of Zephaniah we can see thathe_________.A.was physically a very big manB.preferred the lonely life of a sailorC.always stayed at homeD.was frugal and saved a lotText BBasic to any understanding of Canada in the 20 years after the Second World War is the country' s impressive population growth. For every three Canadians in 1945, there were over five in 1966. In September 1966 Canada's population passed the 20 million mark. Most of this surging growth came from natural increase. The depression of the 1930s and the war had held back marriages, and the catching-up process began after 1945. The baby boom continued through the decade of the 1950s, producing a population increase of nearly fifteen percent in the five years from 1951 to 1956. This rate of increase had been exceeded only once before in Canada's history, in the decade before 1911 when the prairies were being settled. Undoubtedly, the good economic conditions of the 1950s supported a growth in the population, but the expansion also derived from a trend toward earlier marriages and an increase in the average size of families; In 1957 the Canadian birth rate stood at 28 per thousand, one of the highest in the world. After the peak year of 1957, thebirth rate in Canada began to decline. It continued falling until in 1966 it stood at the lowest level in 25 years. Partly this decline reflected the low level of births during the depression and the war, but it was also caused by changes in Canadian society. Young people were staying at school longer, more women were working; young married couples were buying automobiles or houses before starting families; rising living standards were cutting down the size of families. It appeared that Canada was once more falling in step with the trend toward smaller families that had occurred all through theWestern world since the time of the Industrial Revolution. Although the growth in Canada’s population had slowed down by 1966 (the cent), another increase in the first half of the 1960s was only nine percent), another large population wave was coming over the horizon. It would be composed of the children of the children who were born during the period of the high birth rate prior to 1957.6. What does the passage mainly discuss?A. Educational changes in Canadian society.B. Canada during the Second World War.C. Population trends in postwar Canada.D. Standards of living in Canada.7. According to the passage, when did Canada's baby boom begin?A. In the decade after 1911.B. After 1945.C. During the depression of the 1930s.D. In 1966.8. The author suggests that in Canada during the 1950s____________.A. the urban population decreased rapidlyB. fewer people marriedC. economic conditions were poorD. the birth rate was very high9. When was the birth rate in Canada at its lowest postwar level?A. 1966.B. 1957.C. 1956.D. 1951.10. The author mentions all of the following as causes of declines inpopulation growth after 1957 EXCEPT_________________.A. people being better educatedB. people getting married earlierC. better standards of livingD. couples buying houses11.I t can be inferred from the passage that before the IndustrialRevolution_______________.A. families were largerB. population statistics were unreliableC. the population grew steadilyD. economic conditions were badText CI was just a boy when my father brought me to Harlem for the first time, almost 50 years ago. We stayed at the hotel Theresa, a grand brick structure at 125th Street and Seventh avenue. Once, in the hotel restaurant, my father pointed out Joe Louis. He even got Mr. Brown, the hotel manager, to introduce me to him, a bit punchy强力的but still champ焦急as fast as I was concerned.Much has changed since then. Business and real estate are booming. Some say a new renaissance is under way. Others decry责难what they see as outside forces running roughshod肆意践踏over the old Harlem. New York meant Harlem to me, and as a young man I visited it whenever I could. But many of my old haunts are gone. The Theresa shut down in 1966. National chains that once ignored Harlem now anticipate yuppie money and want pieces of this prime Manhattan real estate. So here I am on a hot August afternoon, sitting in a Starbucks that two years ago opened a block away from the Theresa, snatching抓取，攫取at memories between sips of high-priced coffee. I am about to open up a piece of the old Harlem---the New York Amsterdam News---when a tourist。

真空衰减法英语

真空衰减法英语The Vacuum Decay MethodThe vacuum decay method is a fascinating and complex phenomenon in the field of quantum mechanics. This process, also known as false vacuum decay, occurs when the universe transitions from a metastable state to a more stable state, resulting in the release of a large amount of energy. This topic has captured the attention of physicists and cosmologists alike, as it has significant implications for our understanding of the fundamental nature of the universe.At the heart of the vacuum decay process is the concept of the quantum vacuum. In quantum field theory, the vacuum is not simply an empty space but rather a dynamic entity that is filled with virtual particles constantly appearing and disappearing. These virtual particles are the result of the uncertainty principle, which allows for the temporary creation of particle-antiparticle pairs from the vacuum. While these pairs are short-lived, they can have a significant impact on the overall state of the vacuum.The vacuum can exist in different states, known as "true" and "false"vacua. The true vacuum is the state of lowest energy, where the universe is in its most stable configuration. The false vacuum, on the other hand, is a metastable state, where the energy of the system is higher than the true vacuum, but the system is trapped in a local minimum. This metastable state can persist for a long time, but it is inherently unstable and can eventually decay to the true vacuum.The vacuum decay process is triggered by the formation of a bubble of the true vacuum within the false vacuum. This bubble can be thought of as a region where the field configuration has transitioned to the true vacuum state. As this bubble expands, it releases a large amount of energy, which can have dramatic consequences for the surrounding space-time.The rate at which the vacuum decay occurs is determined by the properties of the false vacuum and the potential energy barrier that separates it from the true vacuum. This rate is typically extremely slow, with the lifetime of the false vacuum being many orders of magnitude longer than the current age of the universe. However, under certain conditions, such as the presence of high-energy particles or the application of strong external fields, the rate of vacuum decay can be significantly increased.The implications of the vacuum decay process are far-reaching and have been the subject of extensive research in theoretical physics.One of the most significant consequences is the potential for the universe to undergo a catastrophic phase transition, where the false vacuum suddenly collapses, releasing an enormous amount of energy. This could potentially lead to the destruction of the entire observable universe, as the true vacuum state may be incompatible with the existence of the fundamental particles and forces that we observe.Another implication of the vacuum decay process is its potential impact on the evolution of the universe. If the false vacuum were to decay, it could lead to the formation of new regions of space-time with different physical laws and properties. This could have profound consequences for the structure and evolution of the cosmos, potentially altering the course of cosmic history.Despite the potential dangers of the vacuum decay process, it also holds promise for applications in theoretical physics and cosmology. For example, the study of vacuum decay can provide insights into the nature of the fundamental forces and the structure of the universe at the most fundamental level. Additionally, the energy released during the decay process could potentially be harnessed for technological applications, such as the generation of clean and sustainable energy.In conclusion, the vacuum decay method is a fascinating andcomplex phenomenon that continues to captivate the scientific community. As our understanding of this process deepens, it is likely that we will uncover new and exciting insights into the nature of the universe and the fundamental laws that govern it. Whether the vacuum decay process ultimately leads to the destruction or transformation of the cosmos, it remains a topic of intense interest and ongoing research in the field of theoretical physics.。

cfd中的asymptotic theory -回复

cfd中的asymptotic theory -回复什么是asymptotic theory（渐进理论）？Asymptotic theory（渐进理论）是数学和统计学领域中的一个重要概念，它在研究一系列趋于无穷或者趋于零的变量时起着至关重要的作用。

该理论基于一个核心观点，即当样本的大小趋于无穷大时，统计推断中的一些特征将趋于某个确定的值。

换句话说，渐进理论研究了随着样本规模的增大，估计量、检验统计量和概率分布等数学对象的渐进性质。

为何需要asymptotic theory（渐进理论）？在实际问题中，我们经常需要根据观测数据对未知参数进行估计，进行假设检验或构建置信区间等统计推断。

然而，在许多情况下，由于样本量的限制或数据的特殊性质，准确地计算或推断可能变得非常困难。

此时，渐进理论则可以提供一种方法，使我们能够通过研究样本规模趋于无穷的极限性质，来推导出近似结果。

因此，渐进理论不仅能够简化计算，还可以提供关于估计量和统计检验的渐近理论保证。

渐进理论的核心思想是什么？渐进理论的核心思想是通过分析样本规模逐渐增大时的极限行为，来研究各种统计问题。

有两个关键概念贯穿于整个渐进理论中，分别是渐进效率和渐近正态性。

首先，渐进效率是指当样本规模趋于无穷时，估计量或检验统计量的方差的下界。

也就是说，对于给定的问题，渐进效率能够告诉我们使用不同估计量或检验统计量时，哪种方法能够在渐近意义下给出最小的方差。

其次，渐近正态性是指当样本规模趋于无穷时，统计量（如估计量、检验统计量）的分布将趋近于正态分布。

这意味着，对于大样本问题，我们可以利用正态分布的性质来进行数学推导和近似计算，从而简化问题的复杂度。

如何进行asymptotic theory（渐进理论）的分析？在进行渐进理论的分析时，一般需要经历以下几个步骤：1. 研究给定的统计问题，明确定义要研究的统计量、参数和假设。

2. 推导出给定统计量的极限分布或渐近分布，通常采用中心极限定理、大数定理或其他渐进结果来完成。

2009职称翻译

2-硝氧乙基环氧乙烷，聚2-硝氧乙基环氧乙烷以及制备方法发明背景目前，HTPB一种塑胶炸药（PBX’s）粘合剂的预聚物，已经广泛用于聚氨酯类粘合剂。

这种粘合剂在PBX’s中的用量大约为15%用以提高PBX’s的化学性能。

但是，这种粘合剂是一种钝感粘合剂，会引起PBX’s能量下降。

因此人们做了许多尝试来寻找一种高喊能粘合剂以提高PBX’s的能量。

作为寻找含能年合计的结果，聚缩水甘油醚硝酸酯（PGN）是具有代表性的一个，如图I所示。

PGN的单体结构如图II所示。

但是，如下面反应式1所示，形成聚氨酯弹性体后，反应式1中的PGN会在聚氨酯弹性体中自降解。

像反应式1所示那样，当使用图I中的PGN形成聚氨酯弹性体后，与硝酸酯基团相连的碳上的氢键显示酸性，因此很容易发生反应式1表示的降解反应，因此导致聚氨酯主链降解。

尽管如此，当人们认识到PGN是当前性能最佳的含能预聚后，许多研究都在努力解决这一问题。

但是显著的成果到目前为止还没发现。

详细描述本发明涉及到一系列韩能预聚物用于高喊能顿感的高性能炸药。

特别的，本发明提供了一个新的组分，如图III所示的2-硝氧乙基环氧乙烷和图IV所示的聚2-硝氧乙基环氧乙烷，是由上面的聚合而成。

图III所示的2-硝氧乙基环氧乙烷是组成图IV所示聚2-硝氧乙基环氧乙烷的单体。

另外，聚2-硝氧乙基环氧乙烷有着与反应式1所示的PGN相似的化学结构，后者作为是用于高含能粘合剂的预聚物，同时也表明聚2-硝氧乙基环氧乙烷作为高喊能预聚物的性能也接近PGN。

在反应式1中的PGN的与硝酸酯基团相连的氢与碳形成氢键其不稳定，同时亚氨基攻击不稳定的氢，因此氢被转移到新的位置。

但是，与PGN不同，像反应式2所示那样，本发明的组分IV包含额外一个亚甲基，借以使不稳定的氢不会受到卷纸集团的攻击，因此组分IV不会在聚氨酯弹性体中自降解。

综上所述，组分IV作为高含能预聚物对解决PGN应用中的问题是非常有用的。

一种合成2-硝氧乙基环氧乙烷和合成聚2-硝氧乙基环氧乙烷的方法在下面反应式3中给出：反应是将在下面做进一步的详细阐述。

英语翻译

1`Cutting-Edge Techniques Used for the Structural Investigation of Single Crystals 用于单晶体结构的边缘切割技术的研究X-ray crystallography has become the leading technique for studying the structure of matter at the atomicand molecular level.X射线晶体学已成为在原子和分子水平上研究物质的结构主导技术。

2`Developments in X-ray Crystallographic Structure Determination of Biological Macromolecules 在生物大分子结构测定X射线晶体学的发展The three-dimensional structures of large biomolecules important in the function and mechanistic pathwaysof all living systems and viruses can be determined by x-ray diffraction from crystals of these molecules and their complexes.在所有的生物系统和病毒的作用和机理的途径的重要的生物大分子的三维结构可以用X射线衍射确定从这些分子晶体及其配合物。

3`Femtosecond Crystallography with Ultrabright Electrons and X-rays: Capturing Chemistry in Action飞秒激光晶体以超高亮度电子和X射线：动作捕捉化学With the recent advances in ultrabright electron and x-ray sources, it is now possible to extend crystallography to the femtosecond time domain to literally light up atomic motions involved in the primary processes governing structural transitions.随着近年来超亮电子和X射线源，它现在可以延长晶体飞秒时域上点燃的原子运动参与治理结构变迁的主要过程。

物理学英语词汇_日常英语词汇

bet公式bet formuladlvo理论 dlvo theoryhlb法hydrophile-lipophile balance methodpvt性质 pvt propertyζ电势 zeta potential阿伏加德罗常数avogadro’number阿伏加德罗定律 avogadro law阿累尼乌斯电离理论arrhenius ionization theory阿累尼乌斯方程arrhenius equation阿累尼乌斯活化能 arrhenius activation energy阿马格定律 amagat law艾林方程 erying equation爱因斯坦光化当量定律einstein’s law of photochemical equivalence爱因斯坦－斯托克斯方程 einstein-stokes equation安托万常数 antoine constant安托万方程 antoine equation盎萨格电导理论onsager’s theory of conductance半电池half cell半衰期half time period饱和液体 saturated liquids饱和蒸气 saturated vapor饱和吸附量 saturated extent of adsorption饱和蒸气压 saturated vapor pressure爆炸界限 explosion limits比表面功 specific surface work比表面吉布斯函数 specific surface gibbs function比浓粘度 reduced viscosity标准电动势 standard electromotive force标准电极电势 standard electrode potential标准摩尔反应焓 standard molar reaction enthalpy标准摩尔反应吉布斯函数standard gibbs function of molar reaction标准摩尔反应熵 standard molar reaction entropy标准摩尔焓函数 standard molar enthalpy function标准摩尔吉布斯自由能函数standard molar gibbs free energy function标准摩尔燃烧焓 standard molar combustion enthalpy标准摩尔熵 standard molar entropy标准摩尔生成焓 standard molar formation enthalpy标准摩尔生成吉布斯函数standard molar formation gibbs function标准平衡常数 standard equilibrium constant标准氢电极 standard hydrogen electrode标准态 standard state标准熵 standard entropy标准压力 standard pressure标准状况 standard condition表观活化能apparent activation energy表观摩尔质量 apparent molecular weight表观迁移数apparent transference number表面 surfaces表面过程控制 surface process control表面活性剂surfactants表面吸附量 surface excess表面张力 surface tension表面质量作用定律 surface mass action law波义尔定律 boyle law波义尔温度 boyle temperature波义尔点 boyle point玻尔兹曼常数 boltzmann constant玻尔兹曼分布 boltzmann distribution玻尔兹曼公式 boltzmann formula玻尔兹曼熵定理 boltzmann entropy theorem玻色－爱因斯坦统计bose-einstein statistics泊poise不可逆过程 irreversible process不可逆过程热力学thermodynamics of irreversible processes 不可逆相变化 irreversible phase change布朗运动 brownian movement查理定律charle’s law产率 yield敞开系统 open system超电势 over potential沉降 sedimentation沉降电势 sedimentation potential沉降平衡 sedimentation equilibrium触变 thixotropy粗分散系统 thick disperse system催化剂 catalyst单分子层吸附理论 mono molecule layer adsorption单分子反应 unimolecular reaction单链反应 straight chain reactions弹式量热计 bomb calorimeter道尔顿定律 dalton law道尔顿分压定律 dalton partial pressure law德拜和法尔肯哈根效应debye and falkenhagen effect德拜立方公式 debye cubic formula德拜－休克尔极限公式 debye-huckel’s limiting equation 等焓过程 isenthalpic process等焓线isenthalpic line等几率定理 theorem of equal probability等温等容位helmholtz free energy等温等压位gibbs free energy等温方程 equation at constant temperature低共熔点 eutectic point低共熔混合物 eutectic mixture低会溶点 lower consolute point低熔冰盐合晶 cryohydric第二类永动机 perpetual machine of the second kind第三定律熵 third-law entropy第一类永动机 perpetual machine of the first kind缔合化学吸附 association chemical adsorption电池常数 cell constant电池电动势 electromotive force of cells电池反应 cell reaction电导 conductance电导率 conductivity电动势的温度系数 temperature coefficient of electromotive force电动电势 zeta potential电功electric work电化学 electrochemistry电化学极化 electrochemical polarization电极电势 electrode potential电极反应 reactions on the electrode电极种类 type of electrodes电解池 electrolytic cell电量计 coulometer电流效率current efficiency电迁移 electro migration电迁移率 electromobility电渗 electroosmosis电渗析 electrodialysis电泳 electrophoresis丁达尔效应 dyndall effect定容摩尔热容 molar heat capacity under constant volume 定容温度计 constant voiume thermometer定压摩尔热容 molar heat capacity under constant pressure 定压温度计 constant pressure thermometer定域子系统 localized particle system动力学方程kinetic equations动力学控制 kinetics control独立子系统 independent particle system对比摩尔体积 reduced mole volume对比体积 reduced volume对比温度 reduced temperature对比压力 reduced pressure对称数 symmetry number对行反应reversible reactions对应状态原理 principle of corresponding state多方过程polytropic process多分子层吸附理论adsorption theory of multi-molecular layers二级反应second order reaction二级相变second order phase change法拉第常数 faraday constant法拉第定律faraday’s law反电动势back e.m.f.反渗透 reverse osmosis反应分子数 molecularity反应级数 reaction orders反应进度 extent of reaction反应热heat of reaction反应速率rate of reaction反应速率常数 constant of reaction rate范德华常数 van der waals constant范德华方程 van der waals equation范德华力 van der waals force范德华气体 van der waals gases范特霍夫方程van’t hoff equation范特霍夫规则van’t hoff rule范特霍夫渗透压公式van’t hoff equation of osmotic pressure非基元反应 non-elementary reactions非体积功 non-volume work非依时计量学反应time independent stoichiometric reactions菲克扩散第一定律fick’s first law of diffusion沸点 boiling point沸点升高 elevation of boiling point费米－狄拉克统计fermi-dirac statistics分布 distribution分布数 distribution numbers分解电压 decomposition voltage分配定律 distribution law分散系统 disperse system分散相 dispersion phase分体积 partial volume分体积定律 partial volume law分压 partial pressure分压定律 partial pressure law分子反应力学 mechanics of molecular reactions分子间力 intermolecular force分子蒸馏molecular distillation封闭系统 closed system附加压力 excess pressure弗罗因德利希吸附经验式freundlich empirical formula of adsorption负极 negative pole负吸附 negative adsorption复合反应composite reaction盖·吕萨克定律 gay-lussac law盖斯定律 hess law甘汞电极 calomel electrode感胶离子序 lyotropic series杠杆规则 lever rule高分子溶液 macromolecular solution高会溶点 upper consolute point隔离法the isolation method格罗塞斯－德雷珀定律 grotthus-draoer’s law隔离系统 isolated system根均方速率 root-mean-square speed功 work功函work content共轭溶液 conjugate solution共沸温度 azeotropic temperature构型熵configurational entropy孤立系统 isolated system固溶胶 solid sol固态混合物 solid solution固相线 solid phase line光反应 photoreaction光化学第二定律 the second law of actinochemistry光化学第一定律 the first law of actinochemistry光敏反应 photosensitized reactions光谱熵 spectrum entropy广度性质 extensive property广延量 extensive quantity广延性质 extensive property规定熵 stipulated entropy过饱和溶液 oversaturated solution过饱和蒸气 oversaturated vapor过程 process过渡状态理论 transition state theory过冷水 super-cooled water过冷液体 overcooled liquid过热液体 overheated liquid亥姆霍兹函数 helmholtz function亥姆霍兹函数判据 helmholtz function criterion亥姆霍兹自由能 helmholtz free energy亥氏函数 helmholtz function焓 enthalpy亨利常数 henry constant亨利定律 henry law恒沸混合物 constant boiling mixture恒容摩尔热容 molar heat capacity at constant volume 恒容热 heat at constant volume恒外压 constant external pressure恒压摩尔热容 molar heat capacity at constant pressure恒压热 heat at constant pressure化学动力学chemical kinetics化学反应计量式stoichiometric equation of chemical reaction化学反应计量系数stoichiometric coefficient of chemical reaction化学反应进度 extent of chemical reaction化学亲合势 chemical affinity化学热力学chemical thermodynamics化学势 chemical potential化学势判据 chemical potential criterion化学吸附 chemisorptions环境 environment环境熵变 entropy change in environment挥发度volatility混合熵 entropy of mixing混合物 mixture活度 activity活化控制 activation control活化络合物理论 activated complex theory活化能activation energy霍根-华森图 hougen-watson chart基态能级 energy level at ground state基希霍夫公式 kirchhoff formula基元反应elementary reactions积分溶解热 integration heat of dissolution吉布斯－杜亥姆方程 gibbs-duhem equation吉布斯－亥姆霍兹方程 gibbs-helmhotz equation吉布斯函数 gibbs function吉布斯函数判据 gibbs function criterion吉布斯吸附公式gibbs adsorption formula吉布斯自由能 gibbs free energy吉氏函数 gibbs function极化电极电势 polarization potential of electrode 极化曲线 polarization curves极化作用 polarization极限摩尔电导率 limiting molar conductivity几率因子 steric factor计量式 stoichiometric equation计量系数 stoichiometric coefficient价数规则 rule of valence简并度 degeneracy键焓bond enthalpy胶冻 broth jelly胶核 colloidal nucleus胶凝作用 demulsification胶束micelle胶体 colloid胶体分散系统 dispersion system of colloid胶体化学 collochemistry胶体粒子 colloidal particles胶团 micelle焦耳joule焦耳-汤姆生实验 joule-thomson experiment焦耳-汤姆生系数 joule-thomson coefficient焦耳-汤姆生效应 joule-thomson effect焦耳定律 joule`s law接触电势contact potential接触角 contact angle节流过程 throttling process节流膨胀 throttling expansion节流膨胀系数 coefficient of throttling expansion结线 tie line结晶热heat of crystallization解离化学吸附 dissociation chemical adsorption界面 interfaces界面张力 surface tension浸湿 immersion wetting浸湿功 immersion wetting work精馏 rectify聚（合）电解质polyelectrolyte聚沉 coagulation聚沉值 coagulation value绝对反应速率理论 absolute reaction rate theory绝对熵 absolute entropy绝对温标absolute temperature scale绝热过程 adiabatic process绝热量热计adiabatic calorimeter绝热指数 adiabatic index卡诺定理 carnot theorem卡诺循环 carnot cycle开尔文公式 kelvin formula柯诺瓦洛夫－吉布斯定律 konovalov-gibbs law科尔劳施离子独立运动定律kohlrausch’s law of independent migration of ions可能的电解质potential electrolyte可逆电池 reversible cell可逆过程 reversible process可逆过程方程 reversible process equation可逆体积功 reversible volume work可逆相变 reversible phase change克拉佩龙方程 clapeyron equation克劳修斯不等式 clausius inequality克劳修斯－克拉佩龙方程 clausius-clapeyron equation控制步骤 control step库仑计 coulometer扩散控制 diffusion controlled拉普拉斯方程laplace’s equation拉乌尔定律 raoult law兰格缪尔－欣谢尔伍德机理langmuir-hinshelwood mechanism兰格缪尔吸附等温式 langmuir adsorption isotherm formula 雷利公式 rayleigh equation冷冻系数coefficient of refrigeration冷却曲线 cooling curve离解热heat of dissociation离解压力dissociation pressure离域子系统 non-localized particle systems离子的标准摩尔生成焓 standard molar formation of ion离子的电迁移率 mobility of ions离子的迁移数transport number of ions离子独立运动定律 law of the independent migration of ions 离子氛 ionic atmosphere离子强度 ionic strength理想混合物 perfect mixture理想气体 ideal gas接触电势contact potential接触角 contact angle节流过程 throttling process节流膨胀 throttling expansion节流膨胀系数 coefficient of throttling expansion结线 tie line结晶热heat of crystallization解离化学吸附 dissociation chemical adsorption界面 interfaces界面张力 surface tension浸湿 immersion wetting浸湿功 immersion wetting work精馏 rectify聚（合）电解质polyelectrolyte聚沉 coagulation聚沉值 coagulation value绝对反应速率理论 absolute reaction rate theory绝对熵 absolute entropy绝对温标absolute temperature scale绝热过程 adiabatic process绝热量热计adiabatic calorimeter绝热指数 adiabatic index卡诺定理 carnot theorem卡诺循环 carnot cycle开尔文公式 kelvin formula柯诺瓦洛夫－吉布斯定律 konovalov-gibbs law科尔劳施离子独立运动定律kohlrausch’s law of independent migration of ions可能的电解质potential electrolyte可逆电池 reversible cell可逆过程 reversible process可逆过程方程 reversible process equation可逆体积功 reversible volume work可逆相变 reversible phase change克拉佩龙方程 clapeyron equation克劳修斯不等式 clausius inequality克劳修斯－克拉佩龙方程 clausius-clapeyron equation控制步骤 control step库仑计 coulometer扩散控制 diffusion controlled拉普拉斯方程laplace’s equation拉乌尔定律 raoult law兰格缪尔－欣谢尔伍德机理langmuir-hinshelwood mechanism兰格缪尔吸附等温式 langmuir adsorption isotherm formula 雷利公式 rayleigh equation冷冻系数coefficient of refrigeration冷却曲线 cooling curve离解热heat of dissociation离解压力dissociation pressure离域子系统 non-localized particle systems离子的标准摩尔生成焓 standard molar formation of ion离子的电迁移率 mobility of ions离子的迁移数transport number of ions离子独立运动定律 law of the independent migration of ions 离子氛 ionic atmosphere离子强度 ionic strength理想混合物 perfect mixture理想气体 ideal gas理想气体的绝热指数 adiabatic index of ideal gases理想气体的微观模型 micro-model of ideal gas理想气体反应的等温方程isothermal equation of ideal gaseous reactions理想气体绝热可逆过程方程adiabatic reversible processequation of ideal gases理想气体状态方程 state equation of ideal gas理想稀溶液 ideal dilute solution理想液态混合物 perfect liquid mixture粒子 particles粒子的配分函数 partition function of particles连串反应consecutive reactions链的传递物 chain carrier链反应 chain reactions量热熵 calorimetric entropy量子统计quantum statistics量子效率 quantum yield临界参数 critical parameter临界常数 critical constant临界点 critical point临界胶束浓度critical micelle concentration临界摩尔体积 critical molar volume临界温度 critical temperature临界压力 critical pressure临界状态 critical state零级反应zero order reaction流动电势 streaming potential流动功 flow work笼罩效应 cage effect路易斯－兰德尔逸度规则 lewis-randall rule of fugacity 露点 dew point露点线 dew point line麦克斯韦关系式 maxwell relations麦克斯韦速率分布 maxwell distribution of speeds麦克斯韦能量分布 maxwelidistribution of energy毛细管凝结 condensation in capillary毛细现象 capillary phenomena米凯利斯常数 michaelis constant摩尔电导率 molar conductivity摩尔反应焓 molar reaction enthalpy摩尔混合熵 mole entropy of mixing摩尔气体常数 molar gas constant摩尔热容 molar heat capacity摩尔溶解焓 mole dissolution enthalpy摩尔稀释焓 mole dilution enthalpy内扩散控制 internal diffusions control内能 internal energy内压力 internal pressure能级 energy levels能级分布 energy level distribution能量均分原理 principle of the equipartition of energy 能斯特方程 nernst equation能斯特热定理 nernst heat theorem凝固点 freezing point凝固点降低 lowering of freezing point凝固点曲线 freezing point curve凝胶 gelatin凝聚态 condensed state凝聚相 condensed phase浓差超电势 concentration over-potential浓差极化 concentration polarization浓差电池 concentration cells帕斯卡pascal泡点 bubble point泡点线 bubble point line配分函数 partition function配分函数的析因子性质 property that partition function to be expressed as a product of the separate partition functions for each kind of state碰撞截面 collision cross section碰撞数 the number of collisions偏摩尔量 partial mole quantities平衡常数（理想气体反应）equilibrium constants for reactions of ideal gases平动配分函数 partition function of translation平衡分布 equilibrium distribution平衡态 equilibrium state平衡态近似法 equilibrium state approximation平衡状态图 equilibrium state diagram平均活度 mean activity平均活度系统 mean activity coefficient平均摩尔热容 mean molar heat capacity平均质量摩尔浓度 mean mass molarity平均自由程mean free path平行反应parallel reactions破乳 demulsification铺展 spreading普遍化范德华方程 universal van der waals equation其它功 the other work气化热heat of vaporization气溶胶 aerosol气体常数 gas constant气体分子运动论 kinetic theory of gases气体分子运动论的基本方程 foundamental equation of kinetic theory of gases气溶胶 aerosol气相线 vapor line迁移数 transport number潜热latent heat强度量 intensive quantity强度性质 intensive property亲液溶胶 hydrophilic sol氢电极 hydrogen electrodes区域熔化zone melting热 heat热爆炸 heat explosion热泵 heat pump热功当量mechanical equivalent of heat热函heat content热化学thermochemistry热化学方程thermochemical equation热机 heat engine热机效率 efficiency of heat engine热力学 thermodynamics热力学第二定律 the second law of thermodynamics热力学第三定律 the third law of thermodynamics热力学第一定律 the first law of thermodynamics热力学基本方程 fundamental equation of thermodynamics 热力学几率 thermodynamic probability热力学能 thermodynamic energy热力学特性函数characteristic thermodynamic function热力学温标thermodynamic scale of temperature热力学温度thermodynamic temperature热熵thermal entropy热效应heat effect熔点曲线 melting point curve熔化热heat of fusion溶胶 colloidal sol溶解焓 dissolution enthalpy溶液 solution溶胀 swelling乳化剂 emulsifier乳状液 emulsion润湿 wetting润湿角 wetting angle萨克尔－泰特洛德方程 sackur-tetrode equation 三相点 triple point三相平衡线 triple-phase line熵 entropy熵判据 entropy criterion熵增原理 principle of entropy increase渗透压 osmotic pressure渗析法 dialytic process生成反应 formation reaction升华热heat of sublimation实际气体 real gas舒尔采－哈迪规则 schulze-hardy rule松驰力relaxation force松驰时间time of relaxation速度常数reaction rate constant速率方程rate equations速率控制步骤rate determining step塔费尔公式 tafel equation态－态反应 state-state reactions唐南平衡 donnan equilibrium淌度 mobility特鲁顿规则 trouton rule特性粘度 intrinsic viscosity体积功 volume work统计权重 statistical weight统计热力学 statistic thermodynamics统计熵 statistic entropy途径 path途径函数 path function外扩散控制 external diffusion control完美晶体 perfect crystalline完全气体 perfect gas微观状态 microstate微态 microstate韦斯顿标准电池 weston standard battery维恩效应wien effect维里方程 virial equation维里系数 virial coefficient稳流过程 steady flow process稳态近似法 stationary state approximation无热溶液athermal solution无限稀溶液 solutions in the limit of extreme dilution 物理化学 physical chemistry物理吸附 physisorptions吸附 adsorption吸附等量线 adsorption isostere吸附等温线 adsorption isotherm吸附等压线 adsorption isobar吸附剂 adsorbent吸附量 extent of adsorption吸附热 heat of adsorption吸附质 adsorbate析出电势 evolution or deposition potential析因子性质 property that partition function to be expressed as a product of the separate partition functions for each kind of state稀溶液的依数性 colligative properties of dilute solutions稀释焓 dilution enthalpy系统 system系统点 system point系统的环境 environment of system相 phase相变 phase change相变焓 enthalpy of phase change相变化 phase change相变热 heat of phase change相点 phase point相对挥发度relative volatility相对粘度 relative viscosity相律 phase rule相平衡热容heat capacity in phase equilibrium相图 phase diagram相倚子系统 system of dependent particles悬浮液 suspension循环过程 cyclic process压力商 pressure quotient压缩因子 compressibility factor压缩因子图 diagram of compressibility factor亚稳状态 metastable state盐桥 salt bridge盐析 salting out阳极 anode杨氏方程young’s equation液体接界电势 liquid junction potential液相线 liquid phase lines一级反应first order reaction一级相变first order phase change依时计量学反应 time dependent stoichiometric reactions 逸度 fugacity逸度系数 coefficient of fugacity阴极 cathode荧光 fluorescence永动机 perpetual motion machine永久气体 permanent gas有效能 available energy原电池 primary cell原盐效应 salt effect增比粘度 specific viscosity憎液溶胶 lyophobic sol沾湿 adhesional wetting沾湿功 the work of adhesional wetting真溶液 true solution真实电解质real electrolyte真实气体 real gas真实迁移数true transference number振动配分函数 partition function of vibration振动特征温度 characteristic temperature of vibration蒸气压下降 depression of vapor pressure正常沸点 normal point正吸附 positive adsorption支链反应 branched chain reactions直链反应 straight chain reactions指前因子 pre-exponential factor质量作用定律mass action law制冷系数coefficient of refrigeration中和热heat of neutralization轴功 shaft work转动配分函数 partition function of rotation转动特征温度 characteristic temperature of vibration 转化率 convert ratio转化温度conversion temperature状态 state状态方程 state equation状态分布 state distribution状态函数 state function准静态过程quasi-static process准一级反应 pseudo first order reaction自动催化作用 auto-catalysis自由度 degree of freedom自由度数 number of degree of freedom自由焓free enthalpy自由能free energy自由膨胀free expansion组分数 component number最低恒沸点 lower azeotropic point最高恒沸点 upper azeotropic point最佳反应温度 optimal reaction temperature最可几分布 most probable distribution最可几速率 most propable speed。