Accuracy of spherical harmonic approximations for images of lambertian objects under far an
微积分calculus英文单词

微积分英语单词Absolute convergence :绝对收敛Absolute extreme values :绝对极值Absolute maximum and minimum :绝对极大与极小Absolute value :绝对值Absolute value function :绝对值函数Acceleration :加速度Antiderivative :反导数Approximate integration :近似积分Approximation :逼近法Arc length :弧长Area :面积Asymptote :渐近线Average speed :平均速率Average velocity :平均速度Axes, coordinate :坐标轴Axes of ellipse :椭圆之轴at a point :在一点处之连续性as the slope of a tangent :导数看成切线之斜率by differentials :用微分逼近between curves :曲线间之面积Binomial series :二项级数Cartesian coordinates :笛卡儿坐标一般指直角坐标Cartesian coordinates system :笛卡儿坐标系Cauch’s Mean Value Theorem :柯西均值定理Chain Rule :连锁律Change of variables :变数变换Circle :圆Circular cylinder :圆柱Closed interval :封闭区间Coefficient :系数Composition of function :函数之合成Compound interest :复利Concavity :凹性Conchoid :蚌线Cone :圆锥Constant function :常数函数Constant of integration :积分常数Continuity :连续性Continuous function :连续函数Convergence :收敛Coordinate :s :坐标Cartesian :笛卡儿坐标cylindrical :柱面坐标Coordinate axes :坐标轴Coordinate planes :坐标平面Cosine function :余弦函数Critical point :临界点Cubic function :三次函数Curve :曲线Cylinder :圆柱Cylindrical Coordinates :圆柱坐标Distance :距离Divergence :发散Domain :定义域Dot product :点积Double integral :二重积分Decreasing function :递减函数Decreasing sequence :递减数列Definite integral :定积分Degree of a polynomial :多项式之次数Density :密度Derivative :导数Determinant :行列式Differentiable function :可导函数Differential :微分Differential equation :微分方程Differentiation :求导法Directional derivatives :方向导数Discontinuity :不连续性Disk method :圆盘法domain of :导数之定义域differential :微分学Ellipse :椭圆Ellipsoid :椭圆体Epicycloid :外摆线Equation :方程式Even function :偶函数Expected Valued :期望值Exponential Function :指数函数Exponents , laws of :指数率Extreme value :极值Extreme Value Theorem :极值定理Factorial :阶乘First Derivative Test :一阶导数试验法First octant :第一卦限Focus :焦点Fractions :分式Function :函数Fundamental Theorem of Calculus :微积分基本定理from the left :左连续from the right :右连续Geometric series :几何级数Gradient :梯度Graph :图形Green Formula :格林公式Half-angle formulas :半角公式Harmonic series :调和级数Helix :螺旋线Higher Derivative :高阶导数Horizontal asymptote :水平渐近线Horizontal line :水平线Hyperbola :双曲线Hyperboloid :双曲面horizontal :水平渐近线Implicit differentiation :隐求导法Implicit function :隐函数Improper integral :瑕积分Increasing/Decreasing Test :递增或递减试验法Increment :增量Increasing Function :增函数Indefinite integral :不定积分Independent variable :自变数Indeterminate from :不定型Inequality :不等式Infinite point :无穷极限Infinite series :无穷级数Inflection point :反曲点Instantaneous velocity :瞬时速度Integer :整数Integral :积分Integrand :被积分式Integration :积分Integration by part :分部积分法Intercepts :截距Intermediate value of Theorem :中间值定理Interval :区间Inverse function :反函数Inverse trigonometric function :反三角函数Iterated integral :逐次积分integral :积分学implicit :隐求导法Laplace transform :Leplace 变换Law of Cosines :余弦定理Least upper bound :最小上界Left-hand derivative :左导数Left-hand limit :左极限Lemniscate :双钮线Length :长度Level curve :等高线L'Hospital's rule :洛必达法则Limacon :蚶线Limit :极限Linear approximation:线性近似Linear equation :线性方程式Linear function :线性函数Linearity :线性Linearization :线性化Line in the plane :平面上之直线Line in space :空间之直线Lobachevski geometry :罗巴切夫斯基几何Local extremum :局部极值Local maximum and minimum :局部极大值与极小值Logarithm :对数Logarithmic function :对数函数linear :线性逼近法Maximum and minimum values :极大与极小值Mean Value Theorem :均值定理Multiple integrals :重积分Multiplier :乘子Natural exponential function :自然指数函数Natural logarithm function :自然对数函数Natural number :自然数Normal line :法线Normal vector :法向量Number :数of a function :函数之连续性on an interval :在区间之连续性Octant :卦限Odd function :奇函数One-sided limit :单边极限Open interval :开区间Optimization problems :最佳化问题Order :阶Ordinary differential equation :常微分方程 Origin :原点Orthogonal :正交的Parabola :拋物线Parabolic cylinder :抛物柱面Paraboloid :抛物面Parallelepiped :平行六面体Parallel lines :并行线Parameter :参数Partial derivative :偏导数Partial differential equation :偏微分方程 Partial fractions :部分分式Partial integration :部分积分Partiton :分割Period :周期Periodic function :周期函数Perpendicular lines :垂直线Piecewise defined function :分段定义函数 Plane :平面Point of inflection :反曲点Polar axis :极轴Polar coordinate :极坐标Polar equation :极方程式Pole :极点Polynomial :多项式Positive angle :正角Point-slope form :点斜式Power function :幂函数Product :积polar :极坐标partial :偏导数partial :偏微分方程partial :偏微分法Quadrant :象限Quotient Law of limit :极限的商定律Quotient Rule :商定律rectangular :直角坐标Radius of convergence :收敛半径Range of a function :函数的值域Rate of change :变化率Rational function :有理函数Rationalizing substitution :有理代换法Rationalizing substitution :有理代换法Rational number :有理数Real number :实数Rectangular coordinates :直角坐标Rectangular coordinate system :直角坐标系Relative maximum and minimum :相对极大值与极小值Revenue function :收入函数Revolution, solid of :旋转体Revolution, surface of :旋转曲面Riemann Sum :黎曼和Riemannian geometry :黎曼几何Right-hand derivative :右导数Right-hand limit :右极限Root :根Saddle point :鞍点Scalar :纯量Secant line :割线Second derivative :二阶导数Second Derivative Test :二阶导数试验法Second partial derivative :二阶偏导数Sector :扇形Sequence :数列Series :级数Set :集合Shell method :剥壳法Sine function :正弦函数Singularity :奇点Slant asymptote :斜渐近线Slope :斜率Slope-intercept equation of a line :直线的斜截式Smooth curve :平滑曲线Smooth surface :平滑曲面Solid of revolution :旋转体Space :空间Speed :速率Spherical coordinates :球面坐标Squeeze Theorem :夹挤定理Step function :阶梯函数Strictly decreasing :严格递减Strictly increasing :严格递增Sum :和Surface :曲面Surface integral :面积分Surface of revolution :旋转曲面Symmetry :对称slant :斜渐近线spherical :球面坐标Tangent function :正切函数Tangent line :切线Tangent plane :切平面Tangent vector :切向量Total differential :全微分Trigonometric function :三角函数Trigonometric integrals :三角积分Trigonometric substitutions :三角代换法Tripe integrals :三重积分term by term :逐项求导法under a curve :曲线下方之面积vertical :垂直渐近线Value of function :函数值Variable :变数Vector :向量Velocity :速度Vertical asymptote :垂直渐近线Volume :体积X-axis :x 轴x-coordinate :x 坐标x-intercept :x 截距Zero vector :函数的零点Zeros of a polynomial :多项式的零点。
南开大学光学工程专业英语重点词汇汇总

光学专业英语部分refraction [rɪˈfrækʃn]n.衍射reflection [rɪˈflekʃn]n.反射monolayer['mɒnəleɪə]n.单层adj.单层的ellipsoid[ɪ'lɪpsɒɪd]n.椭圆体anisotropic[,ænaɪsə(ʊ)'trɒpɪk]adj.非均质的opaque[ə(ʊ)'peɪk]adj.不透明的;不传热的;迟钝的asymmetric[,æsɪ'metrɪk]adj.不对称的;非对称的intrinsic[ɪn'trɪnsɪk]adj.本质的,固有的homogeneous[,hɒmə(ʊ)'dʒiːnɪəs;-'dʒen-] adj.均匀的;齐次的;同种的;同类的,同质的incidentlight入射光permittivity[,pɜːmɪ'tɪvɪtɪ]n.电容率symmetric[sɪ'metrɪk]adj.对称的;匀称的emergentlight出射光;应急灯.ultrafast[,ʌltrə'fɑ:st,-'fæst]adj.超快的;超速的uniaxial[,juːnɪ'æksɪəl]adj.单轴的paraxial[pə'ræksɪəl]adj.旁轴的;近轴的periodicity[,pɪərɪə'dɪsɪtɪ]n.[数]周期性;频率;定期性soliton['sɔlitɔn]n.孤子,光孤子;孤立子;孤波discrete[dɪ'skriːt]adj.离散的,不连续的convolution[,kɒnvə'luːʃ(ə)n]n.卷积;回旋;盘旋;卷绕spontaneously:[spɒn'teɪnɪəslɪ] adv.自发地;自然地;不由自主地instantaneously:[,instən'teinjəsli]adv.即刻;突如其来地dielectricconstant[ˌdaiiˈlektrikˈkɔnstənt]介电常数,电容率chromatic[krə'mætɪk]adj.彩色的;色品的;易染色的aperture['æpətʃə;-tj(ʊ)ə]n.孔,穴;(照相机,望远镜等的)光圈,孔径;缝隙birefringence[,baɪrɪ'frɪndʒəns]n.[光]双折射radiant['reɪdɪənt]adj.辐射的;容光焕发的;光芒四射的; photomultiplier[,fəʊtəʊ'mʌltɪplaɪə]n.[电子]光电倍增管prism['prɪz(ə)m]n.棱镜;[晶体][数]棱柱theorem['θɪərəm]n.[数]定理;原理convex['kɒnveks]n.凸面体;凸状concave['kɒnkeɪv]n.凹面spin[spɪn]n.旋转;crystal['krɪst(ə)l]n.结晶,晶体;biconical[bai'kɔnik,bai'kɔnikəl] adj.双锥形的illumination[ɪ,ljuːmɪ'neɪʃən] n.照明;[光]照度;approximate[ə'prɒksɪmət] adj.[数]近似的;大概的clockwise['klɒkwaɪz]adj.顺时针方向的exponent[ɪk'spəʊnənt;ek-] n.[数]指数;even['iːv(ə)n]adj.[数]偶数的;平坦的;相等的eigenmoden.固有模式;eigenvalue['aɪgən,væljuː]n.[数]特征值cavity['kævɪtɪ]n.腔;洞,凹处groove[gruːv]n.[建]凹槽,槽;最佳状态;惯例;reciprocal[rɪ'sɪprək(ə)l]adj.互惠的;相互的;倒数的,彼此相反的essential[ɪ'senʃ(ə)l]adj.基本的;必要的;本质的;精华的isotropic[,aɪsə'trɑpɪk]adj,各向同性的;等方性的phonon['fəʊnɒn]n.[声]声子cone[kəʊn]n.圆锥体,圆锥形counter['kaʊntə]n.柜台;对立面;计数器;cutoff['kʌt,ɔːf]n.切掉;中断;捷径adj.截止的;中断的cladding['klædɪŋ]n.包层;interference[ɪntə'fɪər(ə)ns]n.干扰,冲突;干涉borderline['bɔːdəlaɪn]n.边界线,边界;界线quartz[kwɔːts]n.石英droplet['drɒplɪt]n.小滴,微滴precision[prɪ'sɪʒ(ə)n]n.精度,[数]精密度;精确inherently[ɪnˈhɪərəntlɪ]adv.内在地;固有地;holographic[,hɒlə'ɡræfɪk]adj.全息的;magnitude['mægnɪtjuːd]n.大小;量级;reciprocal[rɪ'sɪprək(ə)l]adj.互惠的;相互的;倒数的,彼此相反的stimulated['stimjə,letid]v.刺激(stimulate的过去式和过去分词)cylindrical[sɪ'lɪndrɪkəl]adj.圆柱形的;圆柱体的coordinates[kəu'ɔ:dineits]n.[数]坐标;external[ɪk'stɜːn(ə)l;ek-]n.外部;外观;scalar['skeɪlə]n.[数]标量;discretization[dɪs'kriːtaɪ'zeɪʃən]n.[数]离散化synthesize['sɪnθəsaɪz]vt.合成;综合isotropy[aɪ'sɑtrəpi]n.[物]各向同性;[物]无向性;[矿业]均质性pixel['pɪks(ə)l;-sel]n.(显示器或电视机图象的)像素(passive['pæsɪv]adj.被动的spiral['spaɪr(ə)l]n.螺旋;旋涡;equivalent[ɪ'kwɪv(ə)l(ə)nt]adj.等价的,相等的;同意义的; transverse[trænz'vɜːs;trɑːnz-;-ns-]adj.横向的;横断的;贯轴的;dielectric[,daɪɪ'lektrɪk]adj.非传导性的;诱电性的;n.电介质;绝缘体integral[ˈɪntɪɡrəl]adj.积分的;完整的criteria[kraɪ'tɪərɪə]n.标准,条件(criterion的复数)Dispersion:分散|光的色散spectroscopy[spek'trɒskəpɪ]n.[光]光谱学photovoltaic[,fəʊtəʊvɒl'teɪɪk]adj.[电子]光电伏打的,光电的polar['pəʊlə]adj.极地的;两极的;正好相反的transmittance[trænz'mɪt(ə)ns;trɑːnz-;-ns-] n.[光]透射比;透明度dichroic[daɪ'krəʊɪk]adj.二色性的;两向色性的confocal[kɒn'fəʊk(ə)l]adj.[数]共焦的;同焦点的rotation[rə(ʊ)'teɪʃ(ə)n]n.旋转;循环,轮流photoacoustic[,fəutəuə'ku:stik]adj.光声的exponential[,ekspə'nenʃ(ə)l]adj.指数的;fermion['fɜːmɪɒn]n.费密子(费密系统的粒子)semiconductor[,semɪkən'dʌktə]n.[电子][物]半导体calibration[kælɪ'breɪʃ(ə)n]n.校准;刻度;标度photodetector['fəʊtəʊdɪ,tektə]n.[电子]光电探测器interferometer[,ɪntəfə'rɒmɪtə]n.[光]干涉仪;干涉计static['stætɪk]adj.静态的;静电的;静力的;inverse相反的,反向的,逆的amplified['æmplifai]adj.放大的;扩充的horizontal[hɒrɪ'zɒnt(ə)l]n.水平线,水平面;水平位置longitudinal[,lɒn(d)ʒɪ'tjuːdɪn(ə)l;,lɒŋgɪ-] adj.长度的,纵向的;propagate['prɒpəgeɪt]vt.传播;传送;wavefront['weivfrʌnt]n.波前;波阵面scattering['skætərɪŋ]n.散射;分散telecommunication[,telɪkəmjuːnɪ'keɪʃ(ə)n] n.电讯;[通信]远程通信quantum['kwɒntəm]n.量子论mid-infrared中红外eigenvector['aɪgən,vektə]n.[数]特征向量;本征矢量numerical[njuː'merɪk(ə)l]adj.数值的;数字的ultraviolet[ʌltrə'vaɪələt]adj.紫外的;紫外线的harmonic[hɑː'mɒnɪk]n.[物]谐波。
SphericalHarmonics球谐函数的理解与使用

SphericalHarmonics球谐函数的理解与使用球谐函数(Spherical Harmonics)是用于描述球对称性的函数。
它在数学、物理、计算机图形学等领域中具有广泛的应用。
本文将对球谐函数的理解与使用进行详细介绍。
首先,我们来了解球谐函数的定义。
给定单位球面上的点(x,y,z),球谐函数Yₗⁿ(x,y,z)定义如下:Yₗⁿ(x, y, z) = (-1)^m * sqrt((2ℓ+1)/(4π)*(ℓ-,m,)!/(ℓ+,m,)!)*Pₗ,m,(cosθ)*e^(imφ)其中,Yₗⁿ表示度为ℓ,阶为,m,的球谐函数;ℓ是非负整数,表示球谐函数的度;,m,<=ℓ,m是整数,表示球谐函数的阶;Pₗ,m,(cosθ)是勒让德多项式;θ是点(x, y, z)相对于x轴的极角;φ是点(x, y, z)相对于x轴的方位角;e是自然对数的底。
球谐函数具有下述性质:1.球谐函数是单位球面上的正交基,即不同的球谐函数之间在单位球面上的内积等于0。
2.Yₗⁿ(x,y,z)关于极角θ是奇函数,关于方位角φ是偶函数。
3.在单位球面上,球谐函数Yₗⁿ(x,y,z)的绝对值平方是一个常数,即,Yₗⁿ(x,y,z),²在球面上处处相等。
在物理学中,球谐函数被广泛应用于描述球对称的物理场。
例如,在量子力学中,球谐函数用于描述原子中的电子波函数;在电动力学中,球谐函数用于展开电磁场的球谐分量;在量子力学中,球谐函数用于描述自旋等。
在计算机图形学中,球谐函数也被广泛应用于实时渲染、全局光照以及球形图像处理等领域。
通过将光照场或图像投影到球谐函数系数上,可以实现基于球面光照的实时渲染。
球谐函数还可以用于创建全局光照环境贴图,用于增强场景的真实感。
此外,球谐函数还可以用于球形图像处理,例如球形全景图像的压缩和展开。
值得注意的是,球谐函数展开的精度和复杂度有一定的关系。
一般来说,较高阶的球谐函数能够更准确地近似光照场或图像,但计算复杂度也会增加。
欧盟残余应力标准EN153 05中文翻译稿

EUROPEAN COMMITTEE FOR STANDARDIZATION COMITE EUROEEN DE NORMALISATION EUROPAISCHES KOMITEE FUR NORMUNG
爱派克测试技术(上海)有限公司组织翻译
2
EUROPEAN STANDARD
EN 15305‐2008
EUROPEAN STANDARD
EN 15305‐2008
August 2008
欧盟X射线衍射残余应力测定标准EN 15305-2008中文翻译稿
Non-destructive Testing - Test Method for Residual Stress analysis by X-ray Diffraction
爱派克测试技术(上海)有限公司组织翻译
1
EUROPEAN STANDARD
EN 15305‐2008
August 2008
ICS 19.100
无损检测–用X射线衍射进行残余应力分析 的测试方法
本欧洲标准于2008年7月4日经欧盟标准委员会(CEN)批准。
欧盟标准委员会成员国有义务按照欧盟标准无变更的给予标准国家地位。关于本 标准的最新目录和题录都可从欧盟标准委员会管理中心和欧盟成员国那里得到。
6.2.3 χ 法....................................................... 22
6.2.4 修改的 χ 方法............................................... 23
6.2.5 6.3 6.4 6.5
其它衍射几何............................................... 23 辐射的选择 ................................................. 23 探测器的选择 ............................................... 26 设备的性能 ................................................. 26
Spherical Harmonics

• Use Microsoft DirectX SDK for spherical harmonics computations
Irradiance
• • The radiance of a purely diffuse surface is defined in terms of the surface’s irradiance Irradiance is an integral of the field-radiance function multiplied by the Lambertian cosine term over a hemisphere
[Greger98]
•
Every point in space has a radiance distribution function
– Radiance is a 5D function (3 spacial dimensions and 2 directional dimensions)
Radiance
– Approximates global illumination on the surface – Only for static scenes! – Does not address dynamic objects that move through the scene – Result in beautifully rendered, globally illuminated scenes that contain unrealistic, locally lit dynamic objects
•
Instead! Compress irradiance maps
– Represent each as a vector of spherical harmonic coefficients – Reduces both storage and bandwidth costs
【1】Lambertian Reflectance and Linear Subspaces

Lambertian Reflectance and Linear Subspaces Ronen Basri,Member,IEEE,and David W.Jacobs,Member,IEEE Abstract—We prove that the set of all Lambertian reflectance functions(the mapping from surface normals to intensities)obtained with arbitrary distant light sources lies close to a9D linear subspace.This implies that,in general,the set of images of a convex Lambertian object obtained under a wide variety of lighting conditions can be approximated accurately by a low-dimensional linear subspace,explaining prior empirical results.We also provide a simple analytic characterization of this linear space.We obtain these results byrepresenting lighting using spherical harmonics and describing the effects of Lambertian materials as the analog of a convolution.These results allow us to construct algorithms for object recognition based on linear methods as well as algorithms that use convex optimization to enforce nonnegative lighting functions.We also show a simple way to enforce nonnegative lighting when the images of an object lie near a 4D linear space.We apply these algorithms to perform face recognition by finding the3D model that best matches a2D query image.Index Terms—Face recognition,illumination,Lambertian,linear subspaces,object recognition,specular,spherical harmonics.æ1I NTRODUCTIONV ARIABILITY in lighting has a large effect on the appearance of objects in images,as is illustrated in Fig.1.But we show in this paper that the set of images an object produces under different lighting conditions can,in some cases,be simply characterized as a nine dimensional subspace in the space of all possible images.This characterization can be used to construct efficient recogni-tion algorithms that handle lighting variations.Under normal conditions,light coming from all direc-tions illuminates an object.When the sources of light are distant from an object,we may describe the lighting conditions by specifying the intensity of light as a function of its direction.Light,then,can be thought of as a nonnegative function on the surface of a sphere.This allows us to represent scenes in which light comes from multiple sources,such as a room with a few lamps and, also,to represent light that comes from extended sources, such as light from the sky,or light reflected off a wall.Our analysis begins by representing these lighting func-tions using spherical harmonics.This is analogous to Fourier analysis,but on the surface of the sphere.With this representation,low-frequency light,for example,means light whose intensity varies slowly as a function of direction.To model the way diffuse surfaces turn light into an image,we look at the amount of light reflected as a function of the surface normal(assuming unit albedo),for each lighting condition.We show that these reflectance functions are produced through the analog of a convolution of the lighting function using a kernel that represents Lambert’s reflection. This kernel acts as a low-pass filter with99.2percent of its energy in the first nine components,the zero,first,and second order harmonics.(This part of our analysis was derived independently also by Ramamoorthi and Hanrahan[31].)We use this and the nonnegativity of light to prove that under any lighting conditions,a nine-dimensional linear subspace,for example,accounts for at least98percent of the variability in the reflectance function.This suggests that in general the set of images of a convex,Lambertian object can be approxi-mated accurately by a low-dimensional linear subspace.We further show how to analytically derive this subspace from a model of an object that includes3D structure and albedo.To provide some intuition about these results,consider the example shown in Fig.2.The figure shows a white sphere made of diffuse material,illuminated by three distant lights.The lighting function can be described in this case as the sum of three delta functions.The image of the sphere, however,is smoothly shaded.If we look at a cross-section of the reflectance function,describing how the sphere reflects light,we can see that it is a very smoothed version of three delta functions.The diffuse material acts as a filter,so that the reflected light varies much more slowly than the incoming light.Our results help to explain recent experimental work(e.g., Epstein et al.[10],Hallinan[15],Yuille et al.[40])that has indicated that the set of images produced by an object under a wide range of lighting conditions lies near a low dimensional linear subspace in the space of all possible images.Our results also allow us to better understand several existing recogni-tion methods.For example,previous work showed that,if we restrict every point on the surface of a diffuse object to face every light source(that is,ignoring attached shadows),then the set of images of the object lies in a3D linear space(e.g., Shashua[34]and Moses[26]).Our analysis shows that,in fact, this approach uses the linear space spanned by the three first order harmonics,but omits the significant zeroth order(DC) component.Koenderink and van Doorn[21]augmented this space in order to account for an additional,perfect diffuse component.The additional component in their method is the missing DC component.Our analysis also leads us to new methods of recognizing objects with unknown pose and lighting conditions.In particular,we discuss how the harmonic basis,which is derived analytically from a model of an object,can be used in a linear subspace-based object recognition algorithm,in place.R.Basri is with the Department of Computer Science,The WeizmannInstitute of Science,Rehovot,76100Israel.E-mail:ronen.basri@weizmann.ac.il.. D.W.Jacobs is with NEC Research Institute,4Independence Way,Princeton,NJ08540.E-mail:dwj@.Manuscript received19June2001;revised31Dec.2001;accepted30Apr.2002.Recommended for acceptance by P.Belhumeur.For information on obtaining reprints of this article,please send e-mail to:tpami@,and reference IEEECS Log Number114379.0162-8828/03/$17.00ß2003IEEE Published by the IEEE Computer Societyof a basis derived by performing SVD on large collections of rendered images.Furthermore,we show how we can enforce the constraint that light is nonnegative everywhere by projecting this constraint to the space spanned by the harmonic basis.With this constraint recognition is expressed as a nonnegative least-squares problem that can be solved using convex optimization.This leads to an algorithm for recognizing objects under varying pose and illumination that resembles Georghiades et al.[12],but works in a low-dimensional space that is derived analytically from a model.The use of the harmonic basis,in this case,allows us to rapidly produce a representation to the images of an object in poses determined at runtime.Finally,we discuss the case in which a first order approximation provides an adequate approxima-tion to the images of an object.The set of images then lies near a 4D linear subspace.In this case,we can express the nonnegative lighting constraint analytically.We use this expression to perform recognition in a particularly efficient way,without complex,iterative optimization techniques.The paper is divided as follows:Section 2briefly reviews the relevant studies.Section 3presents our analysis of Lambertian reflectance.Section 4uses this analysis to derive new algorithms for object recognition.Finally,Section 5discusses extensions to specular reflectance.2P AST A PPROACHESOur work is related to a number of recent approaches to object recognition that represent the set of images that an object can produce using low-dimensional linear subspaces of the space of all images.Ullman and Basri [38]analytically derive such a representation for sets of 3D points undergoing scaled orthographic projection.Shashua [34]and Moses [26](see also Nayar and Murase [28]and Zhao and Yang [41])derive a 3D linear representation of the set of images produced by a Lambertian object as lighting changes,but ignoring shadows.Hayakawa [16]uses factorization to build 3D models using this linear representation.Koenderink and van Doorn [21]extend this to a 4D space by allowing the light to include a diffuse component.Our work differs from these in that ourrepresentation accounts for attached shadows.These shadows occur when a surface faces away from a light source.We do not account for cast shadows,which occur when an intervening part of an object blocks the light from reaching a different part of the surface.For convex objects,only attached shadows occur.As is mentioned in Section 1,we show below that the 4D space used by Koenderink and van Doorn is in fact the space obtained by a first order harmonic approximation of the images of the object.The 3D space used by Shashua,Moses,and Hayakawa is the same space,but it lacks the significant DC component.Researchers have collected large sets of images and performed PCA to build representations that capture within class variations (e.g.,Kirby and Sirovich [19],Turk and Pentland [37],and Cootes et al.[7])and variations due to pose and lighting (Murase and Nayar [27],Hallinan [15],Belhumeur et al.[3],and Yuille et al.[40];see also Malzbender et al.[24]).This approach and its variations have been extremely popular in the last decade,particularly in applications to face recognition.Hallinan [15],Epstein et al.[10],and Yuille et al.[40]perform experiments that show that large numbers of images of real,Lambertian objects,taken with varied lighting conditions,do lie near a low-dimensional linear space,justifying this representation.Belhumeur and Kriegman [4]have shown that the set of images of an object under arbitrary illumination forms a convex cone in the space of all possible images.This analysis accounts for attached shadows.In addition,for convex,Lambertian objects,they have shown that this cone (called the illumination cone )may have unbounded dimension.They have further shown how to construct the cone from as few as three images.Georghiades et al.[11],[12]use this representa-tion for object recognition.To simplify the representation (an accurate representation of the illumination cone requires all the images that can be obtained with a single directional source),they further projected the images to low-dimen-sional subspaces obtained by rendering the objects and applying PCA to the rendered images.Our analysis allows us to further simplify this process by using instead the harmonic basis,which is derived analytically from a model of the object.This leads to a significant speed up of the recognition process (see Section 4).Spherical harmonics have been used in graphics to efficiently represent the bidirectional reflection distribution function (BRDF)of different materials by,e.g.,Cabral et al.[6]and Westin et al.[39].Koenderink and van Doorn [20]proposed replacing the spherical harmonics basis with a basis for functions on the half-sphere that is derived from the Zernike polynomials,since BRDFs are defined over a half sphere.Nimeroff et al.[29],Dobashi et al.[8],and Teo et al.Fig.1.The same face,under two different lighting conditions.Fig.2.On the left,a white sphere illuminated by three directional (distant point)sources of light.All the lights are parallel to the image plane,one source illuminates the sphere from above and the two others illuminate the sphere from diagonal directions.In the middle,a cross-section of the lighting function with three peaks corresponding to the three light sources.On the right,a cross-section indicating how the sphere reflects light.We will make precise the intuition that the material acts as a low-pass filtering,smoothing the light as it reflects it.[35]explore specific lighting configurations(e.g.,daylight) that can be represented efficiently as a linear combination of basis lightings.Dobashi et al.[8],in particular,use spherical harmonics to form such a basis.Miller and Hoffman[25]were first to describe the process of turning incoming light into reflection as a convolution. D’Zmura[9]describes this process in terms of spherical harmonics.With this representation,after truncating high order components,the reflection process can be written as a linear transformation and,so,the low-order components of the lighting can be recovered by inverting the transformation. He used this analysis to explore ambiguities in lighting.We extend this work by deriving subspace results for the reflectance function,providing analytic descriptions of the basis images,and constructing new recognition algorithms that use this analysis while enforcing nonnegative lighting.Independent of and contemporaneous with our work, Ramamoorthi and Hanrahan[31],[32],[33]have described the effect of Lambertian reflectance as a convolution and analyzed it in terms of spherical harmonics.Like D’Zmura, they use this analysis to explore the problem of recovering lighting from reflectances.Both the work of Ramamoorthi and Hanrahan and ours(first described in[1])show that Lambertian reflectance acts as a low-pass filter with most of the energy in the first nine components.In addition to this,we show that the space spanned by the first nine harmonics accurately approximates the reflectance function under any light configuration,even when the light is dominated by high frequencies.Furthermore,we show how to use this space for object recognition.Since the first introduction of our work,a number of related papers have further used and extended these ideas in a number of directions.Specifically,Ramamoorthi[30] analyzed the relationship between the principal components of the images produced by an object and the first nine harmonics.Lee et al.[23]constructed approximations to this space using physically realizable lighting.Basri and Jacobs[2] used the harmonic formulation to construct algorithms for photometric stereo under unknown,arbitrary lighting. Finally,Thornber and Jacobs[36]and Ramamoorthi and Hanrahan[32]further examined the effect of specularity and cast shadows.3M ODELING I MAGE F ORMATIONIn this section,we construct an analytically derived repre-sentation of the images produced by a convex,Lambertian object illuminated by distant light sources.We restrict ourselves to convex objects,so we can ignore the effect of shadows cast by one part of the object on another part of it.We assume that the surface of the object reflects light according to Lambert’s law[22],which states that materials absorb light and reflect it uniformly in all directions.The only parameter of this model is the albedo at each point on the object,which describes the fraction of the light reflected at that point.This relatively simple model applies to diffuse(nonshiny) materials.It has been analyzed and used effectively in a number of vision applications.By a“distant”light source we mean that it is valid to make the approximation that a light shines on each point in the scene from the same angle,and with the same intensity(this also rules out,for example,slide projectors).Lighting, however,may come from multiple sources,including diffuse sources such as the sky.We can therefore describe the intensity of the light as a single function of its direction that does not depend on the position in the scene.It is important to note that our analysis accounts for attached shadows,which occur when a point in the scene faces away from a light source.While we are interested in understanding the images created by an object,we simplify this problem by breaking it into two parts.We use an intermediate representation,the reflectance function(also called the reflectance map,see Horn [17,chapters10,11]).Given our assumptions,the amount of light reflected by a white surface patch(a patch with albedo of one)depends on the surface normal at that point,but not on its spatial position.For a specific lighting condition,the reflectance function describes how much light is reflected by each surface normal.In the first part of our analysis,we consider the set of possible reflectance functions produced under different illumination conditions.This analysis is independent of the structure of the particular object we are looking at;it depends only on lighting conditions and the properties of Lambertian reflectance.Then,we discuss the relationship between the reflectance function and the image. This depends on object structure and albedo,but not on lighting,except as it determines the reflectance function.We begin by discussing the relation of lighting and reflectance.Before we proceed,we would like to clarify the relation between the reflectance function and the bidirectional reflection distribution function(BRDF).The BRDF of a surface material is a function that describes the ratio of radiance,the amount of light reflected by the surface in every direction (measured in power per unit area per solid angle),to irradiance,the amount of light falling on the surface in every direction(measured in power per unit area).BRDF is commonly specified in a local coordinate frame,in which the surface normal is fixed at the north pole.The BRDF of a Lambertian surface is constant,since such a surface reflects light equally in all direction,and it is equal to1=%.In contrast, thereflectancefunctiondescribestheradianceofaunitsurface area given the entire distribution of light in the scene.The reflectance function is obtained by integrating the BRDF over all directions of incident light,weighting the intensity of the light by the foreshortening of the surface as seen from each lightsource.Inaddition,thereflectancefunctionisspecifiedin a global,viewer centered coordinate frame in which the viewing direction is fixed at the north pole.For example,if a scene is illuminated by a single directional source(a distant point source)of unit intensity,the reflectance function for every surface normal will contain the appropriate foreshortening of the surface with respect to the light source direction scaled by 1=%.(For surface normals that face away from the light source the reflectance function will vanish.)For simplicity,we omit below the extra factor of1=%that arises from the Lambertian BRDF since it only scales the intensities in the image by a constant factor.3.1Image Formation as the Analog of aConvolutionBoth lighting and reflectance can be described as functions on the surface of the sphere.We describe the intensity of light as a function of its direction.This formulation allows us to consider multiple light sources that illuminate an object simultaneously from many directions.We describe reflec-tance as a function of the direction of the surface normal.To begin,we introduce notation for describing such functions.Let S 2denote the surface of a unit sphere centered at the origin.We will use u;v to denote unit vectors.We denote their Cartesian coordinates as ðx;y;z Þ,with x 2þy 2þz 2¼1.When appropriate,we will denote such vectors by a pair of angles,ð ;0Þ,withu ¼ðx;y;z Þ¼ðcos 0sin ;sin 0sin ;cos Þ;ð1Þwhere 0 %and 0 0 2%.In this coordinate frame,the poles are set at ð0;0;Æ1Þ, denotes the angle between u and ð0;0;1Þ,and it varies with latitude,and 0varies with longitude.We will use ð l ;0l Þto denote a direction of light and ð r ;0r Þto denote a direction of reflectance,although we will drop this subscript when there is no ambiguity.Similarly,we may express the lighting or reflectance directions using unit vectors such as u l or v r .Since we assume that the sphere is illuminated by a distant set of lights all points are illuminated by identical lighting conditions.Consequently,the configuration of lights that illuminate the sphere can be expressed as a nonnegative function ‘ð l ;0l Þ,giving the intensity of the light reaching the sphere from each direction ð l ;0l Þ.We may also write this as ‘ðu l Þ,describing lighting direction with a unit vector.According to Lambert’s law,if a light ray of intensity l and coming from the direction u l reaches a surface point with albedo &and normal direction v r ,then the intensity,i ,reflected by the point due to this light is given byi ¼l ðu l Þ&max ðu l Áv r ;0Þ:ð2ÞIf we fix the lighting,and ignore &for now,then the reflected light is a function of the surface normal alone.We write this function as r ð r ;0r Þ,or r ðv r Þ.If light reaches a point from a multitude of directions,then the light reflected by the point would be the sum of (or in the continuous case the integral over)the contribution for each direction.If we denote k ðu Áv Þ¼max ðu Áv;0Þ,then we can write:r ðv r Þ¼ZS 2k ðu l Áv r Þ‘ðu l Þdu l ;ð3Þwhere RS 2denotes integration over the surface of the sphere.Below,we will occasionally abuse notation and write k ðu Þto denote the max of zero and the cosine of the angle between u and the north pole (that is,omitting v means that v is the north pole).We therefore call k the half-cosine function.We can also write k ð Þ,where is the latitude of u ,since k only depends on the component of u .For any fixed v ,as we vary u (as we do while integrating (3)),then k ðu Áv Þcomputes the half cosine function centered around v instead of the north pole.That is,since v r is fixed inside the integral,we can think of k as a function just of u ,which gives the max of zero and the cosine of the angle between u and v r .Thus,intuitively,(3)is analogous to a convolution,in which we center a kernel (the half-cosine function defined by k ),and integrate its product with a signal (‘).In fact,we will call this a convolution,and writer ðv r Þ¼k ѼdefZ S 2k ðu l Áv r Þ‘ðu l Þdu l :ð4ÞNote that there is some subtlety here since we cannot,ingeneral,speak of convolving a function on the surface of the sphere with an arbitrary kernel.This is because we have three degrees of freedom in how we position a convolution kernel on the surface of the sphere,but the output of theconvolution should be a function on the surface of the sphere,which has only two degrees of freedom.However,since k is rotationally symmetric this ambiguity disappears.In fact,we have been careful to only define convolution for rotationally symmetric k .3.2Spherical Harmonics and the Funk-Hecke TheoremJust as the Fourier basis is convenient for examining the results of convolutions in the plane,similar tools exist for understanding the results of the analog of convolutions on the sphere.We now introduce these tools,and use them to show that in producing reflectance,k acts as a low-pass filter.The surface spherical harmonics are a set of functions that form an orthonormal basis for the set of all functions on the surface of the sphere.We denote these functions by Y nm ,with n ¼0;1;2;...and Àn m n :Y nm ð ;0Þ¼ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffið2n þ1Þ4%ðn Àj m jÞ!ðn þj m jÞ!s P n j m j ðcos Þe im0;ð5Þwhere P nm are the associated Legendre functions ,defined asP nm ðz Þ¼1Àz 2ðÞm=22n !d n þm dz z 2À1ÀÁn :ð6ÞWe say that Y nm is an n th order harmonic.In the course of this paper,it will sometimes be convenient to parameterize Y nm as a function of space coordinates ðx;y;z Þrather than angles.The spherical harmonics,written Y nm ðx;y;z Þ,then become polynomials of degree n in ðx;y;z Þ.The first nine harmonics then becomeY 00¼1ffiffiffiffi4%p Y 10¼ffiffiffiffi34%q z Y e 11¼ffiffiffiffi34%q x Y o11¼ffiffiffiffi34%q yY 20¼12ffiffiffiffi54%q ð3z 2À1ÞY e 21¼3ffiffiffiffiffiffi512%q xz Y o 21¼3ffiffiffiffiffiffi5q yz Y e 22¼3ffiffiffiffiffiffi5q x 2Ày 2ðÞY o 22¼3ffiffiffiffiffiffi512%q xy;ð7Þwhere the superscripts e and o denote the even and the odd components of the harmonics,respectively,(soY nm ¼Y e n j m j ÆiY on j m j ,according to the sign of m ;in fact the even and odd versions of the harmonics are more convenient to use in practice since the reflectance function is real).Because the spherical harmonics form an orthonormal basis,thismeansthatany piecewisecontinuousfunction,f ,on the surface of the sphere can be written as a linear combination of an infinite series of harmonics.Specifically,for any f ,f ðu Þ¼X 1n ¼0X n m ¼Ànf nm Y nm ðu Þ;ð8Þwhere f nm is a scalar value,computed as:f nm ¼ZS 2f ðu ÞY Ãnm ðu Þdu;ð9Þand Y Ãnmðu Þdenotes the complex conjugate of Y nm ðu Þ.If we rotate a function f,this acts as a phase shift.Define for every n the n th order amplitude of f asA n¼defffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi12nþ1X nm¼Ànf2nms:ð10ÞThen,rotating f does not change the amplitude of a particular order.It may shuffle values of the coefficients, f nm,for a particular order,but it does not shift energy between harmonics of different orders.For example, consider a delta function.As in the case of the Fourier transform,the harmonic transform of a delta function has equal amplitude in every order.If the delta function is at the north pole,its transform is nonzero only for the zonal harmonics,in which m¼0.If the delta function is,in general,position,it has some energy in all harmonics.But in either case,the n th order amplitude is the same for all n.Both the lighting function,‘,and the Lambertian kernel, k,can be written as sums of spherical harmonics.Denote by‘¼X1n¼0X nm¼Ànl nm Y nm;ð11Þthe harmonic expansion of‘,and bykðuÞ¼X1n¼0k n Y n0:ð12ÞNote that,because kðuÞis circularly symmetric about the north pole,only the zonal harmonics participate in this expansion,andZ S2kðuÞYÃnmðuÞdu¼0;m¼0:ð13ÞSpherical harmonics are useful in understanding the effect of convolution by k because of the Funk-Hecke theorem, which is analogous to the convolution theorem.Loosely speaking,the theorem states that we can expand‘and k in terms of spherical harmonics and,then,convolving them is equivalent to multiplication of the coefficients of this expansion.We will state the Funk-Hecke theorem here in a form that is specialized to our specific concerns.Our treatment is based on Groemer[13],but Groemer presents a more general discussion in which,for example,the theorem is stated for spaces of arbitrary dimension.Theorem1(Funk-Hecke).Let kðuÁvÞbe a bounded,integrable function on[-1,1].Then:kÃY nm¼ n Y nmwithn¼ffiffiffiffiffiffiffiffiffiffiffiffiffiffi4%rk n:That is,the theorem states that the convolution of a (circularly symmetric)function k with a spherical harmonic Y mn(as defined in(4))results in the same harmonic,scaled by a scalar n. n depends on k and is tied directly to k n,the n th order coefficient of the harmonic expansion of k.Following the Funk-Hecke theorem,the harmonic ex-pansion of the reflectance function,r,can be written as:r¼kѼX1n¼0X nm¼Ànð n l nmÞY nm:ð14ÞThis is the chief implication of the Funk-Hecke theorem for our purposes.3.3Properties of the Convolution KernelThe Funk-Hecke theorem implies that in producing the reflectance function,r,the amplitude of the light,‘,at every order n is scaled by a factor n that depends only on the convolution kernel,k.We can use this to infer analytically what frequencies will dominate r.To achieve this,we treat‘as a signal and k as a filter,and ask how the amplitudes of ‘change as it passes through the filter.The harmonic expansion of the Lambertian kernel(12) can be derived(with some tedious manipulation detailed in Appendix A)yieldingk n¼ffiffi%p2n¼0ffiffi%3pn¼1ðÀ1Þn2þ1ffiffiffiffiffiffiffiffiffiffiffiffiffið2nþ1Þ%pnnn2n!2;even0n!2;odd:8>>>><>>>>:ð15ÞThe first few coefficients,for example,arek0¼ffiffi%p2%0:8862k1¼ffiffi%3p%1:0233k2¼ffiffiffiffi5%p8%0:4954k4¼Àffiffi%p16%À0:1108k6¼ffiffiffiffiffiffi13%p128%0:0499k8¼ffiffiffiffiffiffi17%p256%À0:0285:ð16Þ(k3¼k5¼k7¼0),j k n j approaches zero as OðnÀ2Þ.A graph representation of the coefficients is shown in Fig.3.The energy captured by every harmonic term is measured commonly by the square of its respective coefficient divided by the total squared energy of the transformed function.The total squared energy in the half cosine function is given byZ2%Z%k2ð Þsin d d0¼2%Z%2cos2 sin d ¼2%:ð17ÞFig.3.From left to right:A graph representation of the first11coefficients of the Lambertian kernel,the relative energy captured by each of the coefficients,and the cumulative energy.。
IEEE standard for Terminology and Test methods for ADC Std 1241-2000

IEEE Std1241-2000 IEEE Standard for Terminology and Test Methods for Analog-to-Digital ConvertersSponsorWaveform Measurement and Analysis Technical Committeeof theof theIEEE Instrumentation and Measurement SocietyApproved7December2000IEEE-SA Standards BoardAbstract:IEEE Std1241-2000identifies analog-to-digital converter(ADC)error sources and provides test methods with which to perform the required error measurements.The information in this standard is useful both to manufacturers and to users of ADCs in that it provides a basis for evaluating and comparing existing devices,as well as providing a template for writing specifications for the procurement of new ones.In some applications,the information provided by the tests described in this standard can be used to correct ADC errors, e.g.,correction for gain and offset errors.This standard also presents terminology and definitions to aid the user in defining and testing ADCs.Keywords:ADC,A/D converter,analog-to-digital converter,digitizer,terminology,test methodsThe Institute of Electrical and Electronics Engineers,Inc.3Park Avenue,New York,NY10016-5997,USACopyrightß2001by the Institute of Electrical and Electronics Engineers,Inc.All rights reserved. Published 13 June 2001. Printed in the United States of America.Print:ISBN0-7381-2724-8SH94902PDF:ISBN0-7381-2725-6SS94902No part of this publication may be reproduced in any form,in an electronic retrieval system or otherwise,without the prior written permission of the publisher.IEEE Standards documents are developed within the IEEE Societies and the Standards Coordinating Committees of the IEEE Standards Association(IEEE-SA)Standards Board.The IEEE develops its standards through a consensus development process,approved by the American National Standards Institute,which brings together volunteers representing varied viewpoints and interests to achieve thefinal product.Volunteers are not necessarily members of the Institute and serve without compensation.While the IEEE administers the process and establishes rules to promote fairness in the consensus development process,the IEEE does not independently evaluate,test,or verify the accuracy of any of the information contained in its standards.Use of an IEEE Standard is wholly voluntary.The IEEE disclaims liability for any personal injury,property or other damage,of any nature whatsoever,whether special,indirect,consequential,or compensatory,directly or indirectly resulting from the publication,use of,or reliance upon this,or any other IEEE Standard document.The IEEE does not warrant or represent the accuracy or content of the material contained herein,and expressly disclaims any express or implied warranty,including any implied warranty of merchantability orfitness for a specific purpose,or that the use of the material contained herein is free from patent infringement.IEEE Standards documents are supplied‘‘AS IS.’’The existence of an IEEE Standard does not imply that there are no other ways to produce,test,measure,purchase, market,or provide other goods and services related to the scope of the IEEE Standard.Furthermore,the viewpoint expressed at the time a standard is approved and issued is subject to change brought about through developments in the state of the art and comments received from users of the standard.Every IEEE Standard is subjected to review at least everyfive years for revision or reaffirmation.When a document is more thanfive years old and has not been reaffirmed,it is reasonable to conclude that its contents,although still of some value,do not wholly reflect the present state of the art. Users are cautioned to check to determine that they have the latest edition of any IEEE Standard.In publishing and making this document available,the IEEE is not suggesting or rendering professional or other services for,or on behalf of,any person or entity.Nor is the IEEE undertaking to perform any duty owed by any other person or entity to another.Any person utilizing this,and any other IEEE Standards document,should rely upon the advice of a competent professional in determining the exercise of reasonable care in any given circumstances.Interpretations:Occasionally questions may arise regarding the meaning of portions of standards as they relate to specific applications.When the need for interpretations is brought to the attention of IEEE,the Institute will initiate action to prepare appropriate responses.Since IEEE Standards represent a consensus of concerned interests,it is important to ensure that any interpretation has also received the concurrence of a balance of interests.For this reason, IEEE and the members of its societies and Standards Coordinating Committees are not able to provide an instant response to interpretation requests except in those cases where the matter has previously received formal consideration. Comments for revision of IEEE Standards are welcome from any interested party,regardless of membership affiliation with IEEE.Suggestions for changes in documents should be in the form of a proposed change of text,together with appropriate supporting ments on standards and requests for interpretations should be addressed to:Secretary,IEEE-SA Standards Board445Hoes LaneP.O.Box1331Piscataway,NJ08855-1331USANote:Attention is called to the possibility that implementation of this standard may require use of subjectmatter covered by patent rights.By publication of this standard,no position is taken with respect to theexistence or validity of any patent rights in connection therewith.The IEEE shall not be responsible foridentifying patents for which a license may be required by an IEEE standard or for conducting inquiriesinto the legal validity or scope of those patents that are brought to its attention.IEEE is the sole entity that may authorize the use of certification marks,trademarks,or other designations to indicate compliance with the materials set forth herein.Authorization to photocopy portions of any individual standard for internal or personal use is granted by the Institute of Electrical and Electronics Engineers,Inc.,provided that the appropriate fee is paid to Copyright Clearance Center. To arrange for payment of licensing fee,please contact Copyright Clearance Center,Customer Service,222Rosewood Drive,Danvers,MA01923USA;(978)750-8400.Permission to photocopy portions of any individual standard for educational classroom use can also be obtained through the Copyright Clearance Center.Introduction(This introduction is not a part of IEEE Std1241-2000,IEEE Standard for Terminology and Test Methods for Analog-to-Digital Converters.)This standard defines the terms,definitions,and test methods used to specify,characterize,and test analog-to-digital converters(ADCs).It is intended for the following:—Individuals and organizations who specify ADCs to be purchased—Individuals and organizations who purchase ADCs to be applied in their products —Individuals and organizations whose responsibility is to characterize and write reports on ADCs available for use in specific applications—Suppliers interested in providing high-quality and high-performance ADCs to acquirersThis standard is designed to help organizations and individuals—Incorporate quality considerations during the definition,evaluation,selection,and acceptance of supplier ADCs for operational use in their equipment—Determine how supplier ADCs should be evaluated,tested,and accepted for delivery to end users This standard is intended to satisfy the following objectives:—Promote consistency within organizations in acquiring third-party ADCs from component suppliers—Provide useful practices on including quality considerations during acquisition planning —Provide useful practices on evaluating and qualifying supplier capabilities to meet user requirements—Provide useful practices on evaluating and qualifying supplier ADCs—Assist individuals and organizations judging the quality and suitability of supplier ADCs for referral to end usersSeveral standards have previously been written that address the testing of analog-to-digital converters either directly or indirectly.These include—IEEE Std1057-1994a,which describes the testing of waveform recorders.This standard has been used as a guide for many of the techniques described in this standard.—IEEE Std746-1984[B16]b,which addresses the testing of analog-to-digital and digital-to-analog converters used for PCM television video signal processing.—JESD99-1[B21],which deals with the terms and definitions used to describe analog-to-digital and digital-to-analog converters.This standard does not include test methods.IEEE Std1241-2000for analog-to-digital converters is intended to focus specifically on terms and definitions as well as test methods for ADCs for a wide range of applications.a Information on references can be found in Clause2.b The numbers in brackets correspond to those in the bibliography in Annex C.As of October2000,the working group had the following membership:Steve Tilden,ChairPhilip Green,Secretary&Text EditorW.Thomas Meyer,Figures EditorPasquale Arpaia Giovanni Chiorboli Tom Linnenbrink*B.N.Suresh Babu Pasquale Daponte Solomon MaxAllan Belcher David Hansen Carlo MorandiDavid Bergman Fred Irons Bill PetersonEric Blom Dan Kien Pierre-Yves RoyDan Knierim*Chairman,TC-10CommitteeContributions were also made in prior years by:Jerry Blair John Deyst Norris NahmanWilliam Boyer Richard Kromer Otis M.SolomonSteve Broadstone Yves Langard T.Michael SoudersThe following members of the balloting committee voted on this standard:Pasquale Arpaia Pasquale Daponte W.Thomas MeyerSuresh Babu Philip Green Carlo MorandiEric Blom Fred Irons William E.PetersonSteven Broadstone Dan Knierim Pierre-Yves RoyGiovanni Chiorboli T.E.Linnenbrink Steven J.TildenSolomon MaxWhen the IEEE-SA Standards Board approved this standard on21September2000,it had the following membership:Donald N.Heirman,ChairJames T.Carlo,Vice-ChairJudith Gorman,SecretarySatish K.Aggarwal James H.Gurney James W.MooreMark D.Bowman Richard J.Holleman Robert F.MunznerGary R.Engmann Lowell G.Johnson Ronald C.PetersenHarold E.Epstein Robert J.Kennelly Gerald H.Petersonndis Floyd Joseph L.Koepfinger*John B.PoseyJay Forster*Peter H.Lips Gary S.RobinsonHoward M.Frazier L.Bruce McClung Akio TojoRuben D.Garzon Daleep C.Mohla Donald W.Zipse*Member EmeritusAlso included are the following nonvoting IEEE-SA Standards Board liaisons:Alan Cookson,NIST RepresentativeDonald R.Volzka,TAB RepresentativeDon MessinaIEEE Standards Project EditorContents1.Overview (1)1.1Scope (1)1.2Analog-to-digital converter background (2)1.3Guidance to the user (3)1.4Manufacturer-supplied information (5)2.References (7)3.Definitions and symbols (7)3.1Definitions (7)3.2Symbols and acronyms (14)4.Test methods (18)4.1General (18)4.2Analog input (41)4.3Static gain and offset (43)4.4Linearity (44)4.5Noise(total) (51)4.6Step response parameters (63)4.7Frequency response parameters (66)4.8Differential gain and phase (71)4.9Aperture effects (76)4.10Digital logic signals (78)4.11Pipeline delay (78)4.12Out-of-range recovery (78)4.13Word error rate (79)4.14Differential input specifications (81)4.15Comments on reference signals (82)4.16Power supply parameters (83)Annex A(informative)Comment on errors associated with word-error-rate measurement (84)Annex B(informative)Testing an ADC linearized with pseudorandom dither (86)Annex C(informative)Bibliography (90)IEEE Standard for Terminology and Test Methods for Analog-to-Digital Converters1.OverviewThis standard is divided into four clauses plus annexes.Clause1is a basic orientation.For further investigation,users of this standard can consult Clause2,which contains references to other IEEE standards on waveform measurement and relevant International Standardization Organization(ISO) documents.The definitions of technical terms and symbols used in this standard are presented in Clause3.Clause4presents a wide range of tests that measure the performance of an analog-to-digital converter.Annexes,containing the bibliography and informative comments on the tests presented in Clause4,augment the standard.1.1ScopeThe material presented in this standard is intended to provide common terminology and test methods for the testing and evaluation of analog-to-digital converters(ADCs).This standard considers only those ADCs whose output values have discrete values at discrete times,i.e., they are quantized and sampled.In general,this quantization is assumed to be nominally uniform(the input–output transfer curve is approximately a straight line)as discussed further in 1.3,and the sampling is assumed to be at a nominally uniform rate.Some but not all of the test methods in this standard can be used for ADCs that are designed for non-uniform quantization.This standard identifies ADC error sources and provides test methods with which to perform the required error measurements.The information in this standard is useful both to manufacturers and to users of ADCs in that it provides a basis for evaluating and comparing existing devices,as well as providing a template for writing specifications for the procurement of new ones.In some applications, the information provided by the tests described in this standard can be used to correct ADC errors, e.g.,correction for gain and offset errors.The reader should note that this standard has many similarities to IEEE Std1057-1994.Many of the tests and terms are nearly the same,since ADCs are a necessary part of digitizing waveform recorders.IEEEStd1241-2000IEEE STANDARD FOR TERMINOLOGY AND TEST METHODS 1.2Analog-to-digital converter backgroundThis standard considers only those ADCs whose output values have discrete values at discrete times, i.e.,they are quantized and sampled.Although different methods exist for representing a continuous analog signal as a discrete sequence of binary words,an underlying model implicit in many of the tests in this standard assumes that the relationship between the input signal and the output values approximates the staircase transfer curve depicted in Figure1a.Applying this model to a voltage-input ADC,the full-scale input range(FS)at the ADC is divided into uniform intervals,known as code bins, with nominal width Q.The number of code transition levels in the discrete transfer function is equal to 2NÀ1,where N is the number of digitized bits of the ADC.Note that there are ADCs that are designed such that N is not an integer,i.e.,the number of code transition levels is not an integral power of two. Inputs below thefirst transition or above the last transition are represented by the most negative and positive output codes,respectively.Note,however,that two conventions exist for relating V min and V max to the nominal transition points between code levels,mid-tread and mid-riser.The dotted lines at V min,V max,and(V minþV max)/2indicate what is often called the mid-tread convention,where thefirst transition is Q/2above V min and the last transition is3Q/2,below V max. This convention gets its name from the fact that the midpoint of the range,(V minþV max)/2,occurs in the middle of a code,i.e.,on the tread of the staircase transfer function.The second convention,called the mid-riser convention,is indicated in thefigure by dashed lines at V min,V max,and(V minþV max)/2. In this convention,V min isÀQ from thefirst transition,V max isþQ from the last transition,and the midpoint,(V minþV max)/2,occurs on a staircase riser.The difference between the two conventions is a displacement along the voltage axis by an amount Q/2.For all tests in this standard,this displacement has no effect on the results and either convention may be used.The one place where it does matter is when a device provides or expects user-provided reference signals.In this case the manufacturer must provide the necessary information relating the reference levels to the code transitions.In both conventions the number of code transitions is 2NÀ1and the full-scale range,FSR,is from V min to V max.Even in an ideal ADC,the quantization process produces errors.These errors contribute to the difference between the actual transfer curve and the ideal straight-line transfer curve,which is plotted as a function of the input signal in Figure1b.To use this standard,the user must understand how the transfer function maps its input values to output codewords,and how these output codewords are converted to the code bin numbering convention used in this standard.As shown in Figure1a,the lowest code bin is numbered0, the next is1,and so on up to the highest code bin,numbered(2NÀ1).In addition to unsigned binary(Figure1a),ADCs may use2’s complement,sign-magnitude,Gray,Binary-Coded-Decimal (BCD),or other output coding schemes.In these cases,a simple mapping of the ADC’s consecutive output codes to the unsigned binary codes can be used in applying various tests in this standard.Note that in the case of an ADC whose number of distinct output codes is not an integral power of2(e.g.,a BCD-coded ADC),the number of digitized bits N is still defined,but will not be an integer.Real ADCs have other errors in addition to the nominal quantization error shown in Figure1b.All errors can be divided into the categories of static and dynamic,depending on the rate of change of the input signal at the time of digitization.A slowly varying input can be considered a static signal if its effects are equivalent to those of a constant signal.Static errors,which include the quantization error, usually result from non-ideal spacing of the code transition levels.Dynamic errors occur because of additional sources of error induced by the time variation of the analog signal being sampled.Sources include harmonic distortion from the analog input stages,signal-dependent variations in the time of samples,dynamic effects in internal amplifier and comparator stages,and frequency-dependent variation in the spacing of the quantization levels.1.3Guidance to the user1.3.1InterfacingADCs present unique interfacing challenges,and without careful attention users can experience substandard results.As with all mixed-signal devices,ADCs perform as expected only when the analog and digital domains are brought together in a well-controlled fashion.The user should fully understand the manufacturer’s recommendations with regard to proper signal buffering and loading,input signal connections,transmission line matching,circuit layout patterns,power supply decoupling,and operating conditions.Edge characteristics for start-convert pulse(s)and clock(s)must be carefully chosen to ensure that input signal purity is maintained with sufficient margin up to the analog input pin(s).Most manufacturers now provide excellent ADC evaluation boards,which demonstrate IN P U T IN P U T(a)Figure 1—Staircase ADC transfer function,having full-scale range FSR and 2N À1levels,corresponding to N -bit quantizationIEEE FOR ANALOG-TO-DIGITAL CONVERTERS Std 1241-2000IEEEStd1241-2000IEEE STANDARD FOR TERMINOLOGY AND TEST METHODS recommended layout techniques,signal conditioning,and interfacing for their ADCs.If the characteristics of a new ADC are not well understood,then these boards should be analyzed or used before starting a new layout.1.3.2Test conditionsADC test specifications can be split into two groups:test conditions and test results.Typical examples of the former are:temperature,power supply voltages,clock frequency,and reference voltages. Examples of the latter are:power dissipation,effective number of bits,spurious free dynamic range (SFDR),and integral non-linearity(INL).The test methods defined in this standard describe the measurement of test results for given test conditions.ADC specification sheets will often give allowed ranges for some test condition(e.g.,power supply ranges).This implies that the ADC will function properly and that the test results will fall within their specified ranges for all test conditions within their specified ranges.Since the test condition ranges are generally specified in continuous intervals,they describe an infinite number of possible test conditions,which obviously cannot be exhaustively tested.It is up to the manufacturer or tester of an ADC to determine from design knowledge and/or testing the effect of the test conditions on the test result,and from there to determine the appropriate set of test conditions needed to accurately characterize the range of test results.For example,knowledge of the design may be sufficient to know that the highest power dissipation(test result)will occur at the highest power supply voltage(test condition),so the power dissipation test need be run only at the high end of the supply voltage range to check that the dissipation is within the maximum of its specified range.It is very important that relevant test conditions be stated when presenting test results.1.3.3Test equipmentOne must ensure that the performance of the test equipment used for these tests significantly exceeds the desired performance of the ADC under ers will likely need to include additional signal conditioning in the form offilters and pulse shapers.Accessories such as terminators, attenuators,delay lines,and other such devices are usually needed to match signal levels and to provide signal isolation to avoid corrupting the input stimuli.Quality testing requires following established procedures,most notably those specified in ISO9001: 2000[B18].In particular,traceability of instrumental calibration to a known standard is important. Commonly used test setups are described in4.1.1.1.3.4Test selectionWhen choosing which parameters to measure,one should follow the outline and hints in this clause to develop a procedure that logically and efficiently performs all needed tests on each unique setup. The standard has been designed to facilitate the development of these test procedures.In this standard the discrete Fourier transform(DFT)is used extensively for the extraction of frequency domain parameters because it provides numerous evaluation parameters from a single data record.DFT testing is the most prevalent technique used in the ADC manufacturing community,although the sine-fit test, also described in the standard,provides meaningful data.Nearly every user requires that the ADC should meet or exceed a minimum signal-to-noise-and-distortion ratio(SINAD)limit for the application and that the nonlinearity of the ADC be well understood.Certainly,the extent to whichthis standard is applied will depend upon the application;hence,the procedure should be tailored for each unique characterization plan.1.4Manufacturer-supplied information1.4.1General informationManufacturers shall supply the following general information:a)Model numberb)Physical characteristics:dimensions,packaging,pinoutsc)Power requirementsd)Environmental conditions:Safe operating,non-operating,and specified performance tempera-ture range;altitude limitations;humidity limits,operating and storage;vibration tolerance;and compliance with applicable electromagnetic interference specificationse)Any special or peculiar characteristicsf)Compliance with other specificationsg)Calibration interval,if required by ISO10012-2:1997[B19]h)Control signal characteristicsi)Output signal characteristicsj)Pipeline delay(if any)k)Exceptions to the above parameters where applicable1.4.2Minimum specificationsThe manufacturer shall provide the following specifications(see Clause3for definitions):a)Number of digitized bitsb)Range of allowable sample ratesc)Analog bandwidthd)Input signal full-scale range with nominal reference signal levelse)Input impedancef)Reference signal levels to be appliedg)Supply voltagesh)Supply currents(max,typ)i)Power dissipation(max,typ)1.4.3Additional specificationsa)Gain errorb)Offset errorc)Differential nonlinearityd)Harmonic distortion and spurious responsee)Integral nonlinearityf)Maximum static errorg)Signal-to-noise ratioh)Effective bitsi)Random noisej)Frequency responsek)Settling timel)Transition duration of step response(rise time)m)Slew rate limitn)Overshoot and precursorso)Aperture uncertainty(short-term time-base instability)p)Crosstalkq)Monotonicityr)Hysteresiss)Out-of-range recoveryt)Word error rateu)Common-mode rejection ratiov)Maximum common-mode signal levelw)Differential input impedancex)Intermodulation distortiony)Noise power ratioz)Differential gain and phase1.4.4Critical ADC parametersTable1is presented as a guide for many of the most common ADC applications.The wide range of ADC applications makes a comprehensive listing impossible.This table is intended to be a helpful starting point for users to apply this standard to their particular applications.Table1—Critical ADC parametersTypical applications Critical ADC parameters Performance issuesAudio SINAD,THD Power consumption.Crosstalk and gain matching.Automatic control MonotonicityShort-term settling,long-term stability Transfer function. Crosstalk and gain matching. Temperature stability.Digital oscilloscope/waveform recorder SINAD,ENOBBandwidthOut-of-range recoveryWord error rateSINAD for wide bandwidthamplitude resolution.Low thermal noise for repeatability.Bit error rate.Geophysical THD,SINAD,long-term stability Millihertz response.Image processing DNL,INL,SINAD,ENOBOut-of-range recoveryFull-scale step response DNL for sharp-edge detection. High-resolution at switching rate. Recovery for blooming.Radar and sonar SINAD,IMD,ENOBSFDROut-of-range recovery SINAD and IMD for clutter cancellation and Doppler processing.Spectrum analysis SINAD,ENOBSFDR SINAD and SFDR for high linear dynamic range measurements.Spread spectrum communication SINAD,IMD,ENOBSFDR,NPRNoise-to-distortion ratioIMD for quantization of smallsignals in a strong interferenceenvironment.SFDR for spatialfiltering.NPR for interchannel crosstalk.Telecommunication personal communications SINAD,NPR,SFDR,IMDBit error rateWord error rateWide input bandwidth channel bank.Interchannel crosstalk.Compression.Power consumption.Std1241-2000IEEE STANDARD FOR TERMINOLOGY AND TEST METHODS2.ReferencesThis standard shall be used in conjunction with the following publications.When the following specifications are superseded by an approved revision,the revision shall apply.IEC 60469-2(1987-12),Pulse measurement and analysis,general considerations.1IEEE Std 1057-1994,IEEE Standard for Digitizing Waveform Recorders.23.Definitions and symbolsFor the purposes of this standard,the following terms and definitions apply.The Authoritative Dictionary of IEEE Standards Terms [B15]should be referenced for terms not defined in this clause.3.1Definitions3.1.1AC-coupled analog-to-digital converter:An analog-to-digital converter utilizing a network which passes only the varying ac portion,not the static dc portion,of the analog input signal to the quantizer.3.1.2alternation band:The range of input levels which causes the converter output to alternate between two adjacent codes.A property of some analog-to-digital converters,it is the complement of the hysteresis property.3.1.3analog-to-digital converter (ADC):A device that converts a continuous time signal into a discrete-time discrete-amplitude signal.3.1.4aperture delay:The delay from a threshold crossing of the analog-to-digital converter clock which causes a sample of the analog input to be taken to the center of the aperture for that sample.COMINT ¼communications intelligence DNL ¼differential nonlinearity ENOB ¼effective number of bits ELINT ¼electronic intelligence NPR ¼noise power ratio INL ¼integral nonlinearity DG ¼differential gain errorSIGINT ¼signal intelligenceSINAD ¼signal-to-noise and distortion ratio THD ¼total harmonic distortion IMD ¼intermodulation distortion SFDR ¼spurious free dynamic range DP ¼differential phase errorTable 1—Critical ADC parameters (continued)Typical applicationsCritical ADC parametersPerformance issuesVideoDNL,SINAD,SFDR,DG,DP Differential gain and phase errors.Frequency response.Wideband digital receivers SIGINT,ELINT,COMINTSFDR,IMD SINADLinear dynamic range fordetection of low-level signals in a strong interference environment.Sampling frequency.1IEC publications are available from IEC Sales Department,Case Postale 131,3rue de Varemb,CH 1211,Gen ve 20,Switzerland/Suisse (http://www.iec.ch).IEC publications are also available in the United States from the Sales Department,American National Standards Institute,25W.43rd Street,Fourth Floor,New York,NY 10036,USA ().2IEEE publications are available from the Institute of Electrical and Electronics Engineers,445Hoes Lane,P.O.Box 1331,Piscataway,NJ 08855-1331,USA (/).。
计量经济学中英文词汇对照

Common variance Common variation Communality variance Comparability Comparison of bathes Comparison value Compartment model Compassion Complement of an event Complete association Complete dissociation Complete statistics Completely randomized design Composite event Composite events Concavity Conditional expectation Conditional likelihood Conditional probability Conditionally linear Confidence interval Confidence limit Confidence lower limit Confidence upper limit Confirmatory Factor Analysis Confirmatory research Confounding factor Conjoint Consistency Consistency check Consistent asymptotically normal estimate Consistent estimate Constrained nonlinear regression Constraint Contaminated distribution Contaminated Gausssian Contaminated normal distribution Contamination Contamination model Contingency table Contour Contribution rate Control
Validation of Hybrid MoM Scheme With Included

Validation of Hybrid MoM Scheme With Included Equivalent Glass Antenna Model for HandlingAutomotive EMC ProblemsFaik G.Bogdanov,Member,IEEE,David D.Karkashadze,Member,IEEE,Roman G.Jobava,Member,IEEE, Anna L.Gheonjian,Associate Member,IEEE,Ekaterina A.Yavolovskaya,Natalia G.Bondarenko,and Christoph Ullrich,Student Member,IEEEAbstract—In this paper,based on the refined modified image theory,an equivalent model of the layered glass antenna structure is suggested and validated by comparison with Sommerfeld’s tra-ditional solution to Green’s ing this model,a hybrid method of moments(MoM)scheme is derived to handle the full MoM geometries includingfinite-sized and curved glass antenna structures.This scheme is validated by comparison of the measured and simulated electromagnetic compatibility(EMC)characteris-tics of the special simplified models and a full vehicle model with a realistic rear window glass antenna.Index Terms—Coupling,dielectric substrate,glass antenna, Green’s function,hybrid scheme,image theory,measurements, method of moments(MoM),reflection,simulation,vehicle.I.I NTRODUCTIONA UTOMOTIVE design tends toward conformal and hiddenantenna applications such as glass antennas integrated in vehicle windowpanes[1],as depicted in Fig.1.The rapid in-crease in the number of integrated glass antennas exacerbates demands for electromagnetic compatibility(EMC)of such an-tennas to each other,vehicle wiring,electric equipment,and the body of the vehicle itself.Therefore,development of the proper techniques and computational tools for robust EMC analysis of vehicle glass antennas is in great demand.This initiates intensive numerical modeling and investigation of installed performance of glass antennas on complete vehicle models[2]–[6].An accurate EMC analysis of vehicle glass antennas requires a proper discretization of not only metallic elements,but alsoManuscript received April27,2009;revised September11,2009.First published January12,2010;current version published February18,2010.This work was supported in part by the Antenna Development Department,AUDI AG,Ingolstadt,Germany.F.G.Bogdanov is with the EM Consulting and Software,EMCoS Ltd.,Tbilisi 0160,Georgia,and also with the Department of Telecommunication,Georgian Technical University,Tbilisi0175,Georgia(e-mail:faik.bogdanov@emcos. com).D.D.Karkashadze,R.G.Jobava,A.L.Gheonjian,andE.A.Yavolovskaya are with the EM Consulting and Software,EMCoS Ltd.,Tbilisi0160, Georgia,and also with the Electrical and Electronics Engineering Divi-sion,I.Javakhishvili Tbilisi State University,Tbilisi0128,Georgia(e-mail: david.karkashadze@emcos.ge;roman.jobava@;anna.gheonjian@ ;ekaterina.yavolovskaya@).N.G.Bondarenko was with the EM Consulting and Software,EMCoS Ltd., Tbilisi0160,Georgia.She is now with the Electromagnetic Compatibility Laboratory,Missouri University of Science and Technology,MO65409USA (e-mail:natalia.bondarenko@).C.Ullrich is with AUDI AG,85045Ingolstadt,Germany(e-mail: christoph.ullrich@audi.de).Digital Object Identifier10.1109/TEMC.2009.2036003Fig.1.Vehicle computational model with glass antenna in rear window.the dielectric substrate of glass antennas.Applying the typ-icalfinite-element method/method of moments(FEM/MoM)approach to such a mesh results in excessively large amountof unknowns(a few hundred of thousands).Therefore,somestudies on vehicle glass antennas[2],[3]neglect the windowglazing to be eligible for far-field calculations only.To accountfor dielectric impact of the glass on vehicle EMC characteristics,most of the studies[4]–[6]utilize the sheet impedance approx-imation[7],available in many electromagnetic(EM)solvers,such as[8]–[10].However,although such an approach is ratherefficient,it is not accurate enough for complex glass antenna ge-ometries and still requires discretization of the substrate,thoughappreciably coarser.The discretization of the substrate could be avoided,whenneglecting the curvature of the glass and impact of the mainvehicle geometry,by applying appropriate Green’s functionsfor infinite-layered structures[11]–[14].However,the rigorousGreen’s functions represented by Sommerfeld integrals[15]areunfortunately too time-consuming and inflexible to be incorpo-rated in the full-vehicle geometry.To overcome this problem,some studies[16]–[18]are done to approximate the Sommerfeldintegrals;others propose different approximate image theories,such as the complex image theory(CIT)[19]–[22]and modi-fied image theory(MIT)[23]–[29];thirds suggest the differentequivalent models of layered structures[30]–[33].However,most of such theories and models are suited only for the simple-layered geometries and are notflexible enough to be hybridizedwith full-vehicle geometry.A new simple,but rather accurate andflexible equivalentglass antenna model is reported in[34].It is based on the image 0018-9375/$26.00©2010IEEEconcept,and image amplitudes are expressed through these for dielectric interface problem and obtained by refining the MIT [28],[29].This model is hybridized with main vehicle geometry in[35]and[36].This paper is aimed to:1)expand the theory of equivalent glass antenna model proposed in[34]–[36];2)improve the hy-brid MoM scheme derived in[35]and[36];and3)validate this scheme applied to automotive EMC problems.First,equivalent glass antenna model is validated by comparison of the simu-lated results with Sommerfeld’s traditional solution to Green’s function.Further,the hybrid MoM scheme is validated by com-parison of EMC characteristics of a few hybrid models,included the complex glass antennas with measurement data.Unlike[35] and[36],the glass in these models is not framed,resulting in more complex and sharper resonances.Finally,the hybrid scheme is validated on a full-car model by comparison of the simulated and measured results.The paper is organized as follows.The problem formulation is given in Section II.The proposed theory of the equivalent glass antenna model and the hybrid MoM scheme is presented in Section III.The validation of the equivalent model and the hy-brid MoM scheme is discussed in Section IV.Finally,conclu-sions are given in Section V.II.P ROBLEM F ORMULATIONAs is known,an arbitrary boundary value EM problem on geometry G may be written in operator form asL( J)= g(1) where L is a boundary operator, g is an excitation,and J is an unknown current density on G.To apply the MoM[37]to(1),we discretize the geometry G and consider the following expansion for the unknown current:J( r )=Nn=1I n f n( r )(2)where{ f n( r )}N n=1are the subdomain expansion functions, I n are unknown coefficients,and N is the total number of unknowns.Substituting(2)into(1)and applying a testing procedure with weighting functions{ w m( r)}N m=1defined in the range of L leads to a linear set of equations written in matrix form as[Z m n][I n]=[V m](3) with elements Z m n= w m,L f n and V m= w m, g defining the MoM impedance matrix and excitation column.The described traditional MoM procedure requires discretiza-tion of all parts of geometry,including the dielectric substrate.In order to exclude the dielectric from the formulation,we should modify the boundary operator L and excitation g in the glass area in such a way as to account for the effect of the dielectric and automatically satisfy the boundary conditions on dielectric. Ourfirst aim is tofind the modified boundary operator and ex-citation in the glass area by introducing the equivalent glassan-Fig.2.Original.(a)Equivalent.(b)Glass antenna model.tenna model.Next aim is to incorporate an approximate Green’s function of the equivalent model in a full MoM matrix to ap-ply the hybrid MoM scheme for analysis offinite-sized and curved glass antenna structures integrated into the full-vehicle geometry.Finally,this approach is validated.III.T HEORETICAL A PPROACHA.Equivalent Glass Antenna ModelFig.2(a)shows an original structure of the metallic strip (glass antenna element)A with current J placed above,inside or under the dielectric layer(regions i=1,2,3,respectively). The layer of thickness l and material parametersε,µ(region i= 2)are placed in vacuum with parametersε0,µ0(i=1,3).In a multilayer case,effective medium parameters are considered. Fig.2(b)shows an equivalent model of the microstrip struc-ture of Fig.2(a),consisting of the source current J on element A and its mirror images J k(k=0,±1,±2,...)in the upper and bottom dielectric layer interfaces.For the source current J in the region i,an EMfield at the observation region j=1,2,3is composed of thefield of the original current J(if only j=i)and that produced by its images Jktaken with amplitudes A jikvand A jikhfor vertical and horizon-tal components of the vector potentials,and A jikqfor the scalar potentials.Hereinafter,thefirst superscript in amplitude nota-tions indicates the observation region and the second indicates the source region.Note that both the source and image currents radiate in medium with material properties of the observation region j. Meanwhile,only images that are not situated in the observation region radiate into this region.The suggested model requiresfinding the image amplitudes ensuring the boundary conditions on both dielectric interfaces. This may be done by recursively applying the mirror image method to relate these amplitudes with those obtained for the approximate solution of boundary value problem on separate dielectric interface.In the next section,we refine the so-called MIT[28],[29]to reconsider the dielectric half-space problem in a quasistatic approximation.Further,in Section III-C,we derivethe image amplitudes A jikt,t=v,h,q.B.Refined MITConsider the interface between the two dielectric media m and i characterized by material parametersεm,µm andεi,µi,seeFig.3.Sources and images in the presence of dielectric interface.(a)Original problem.(b)Equivalent problem for the source region i.(c)Equivalent problem for the mirror region m.Fig.3(a).Let the current J and charge q=∓(1/iω)div JdV associated with elementary electric dipole of volume dV exist in one of the two media,say medium i,as depicted in Fig.3(a). We are interested in the calculation of the EMfield due to these sources at the observation points from both sides of the interface.As is known,the aforementioned problem is accurately solved in terms of Sommerfeld integrals.However,to approximately determine thefield in both the source region i and the mirror region m,we take advantage of the MIT[24]–[29],extending the canonical mirror image method to the dielectric half-space problem.Following the MIT,an EM response from the imperfect interface is approximately described by inserting the mirror im-age source radiating to the source region i and space-like image source radiating to mirror region m,see Fig.3(b)and(c),where the original current J is decomposed into its vertical J v and horizontal J h components,and J v=− J v.Unlike the canoni-cal mirror image method,the image amplitudes a ji t(t=v,h,q) are modified as to approximately satisfy boundary conditions (hence the method’s name).The variants of the MIT[23]–[29] differ by the method offinding the image amplitudes.The traditional MIT[23]–[27],also known as the reflection coefficient method(RCM),utilizes the far-field plane-wave ap-proximation for image amplitudes.Therefore,its solution is valid only rather far(kR 1)from the boundary surface,where k is the wavenumber and R is the distance between the image andobservation points.Unlike this,the simplified MIT[28],[29]is obtained in electrostatic approximation,kR 1,and does not consider the boundary conditions for the magneticfield.Thus, the obtained image coefficients are fully eligible only for the charge problem,and the current images are approximately el-igible in the source region for the vertical dipole problem,but fully invalid for the horizontal dipole problem.Therefore,we reconsider this problem again.In order tofind the unknown image amplitudes a ji t(t=v,h,q), we impose the boundary conditions on both electricfield E j=−iω A j−∇Φj and magneticfield H j=(1/µj)∇× A j(j= i,m),due to all sources in their radiation domain considered ina quasi-static approximation(kR)2 1.The electricfield is expressed by means of the sources of both vector and scalar potentials,i.e.,the currents and charges,and magneticfield of only that for vector potential,i.e.,the currents.Imposing the boundary conditions for the same amplitudes of the current and charge images leads to the inconsistent set of equations for image amplitudes.However,we are at liberty to assign the different amplitudes a ji v and a jihfor the current images and a ji q for the charge images,in view of the nonuniqueness of vector and scalar potentials in the presence of a dielectric boundary[38],[39].Furthermore,we consider the vertical and horizontal dipole problems separately,as it is done in traditional Sommerfeld solution.Consequently,we obtain the following set of equations for image amplitudes:1εi(1+a ii q)=1εma m i q1−a ii q=a m i q(4a)1−a ii v=a m i v(4b)1−a ii h=a m i hµi(1+a ii h)=µm a m i h.(4c) Note that(4a)follows from the boundary conditions for elec-tricfield,and(4b)and(4c)for the magneticfield.Therefore,(4) defines an approximate EM solution of Sommerfeld problem. Analysis of(4)shows that a vertical dipole problem has a definite solution only for the same amplitudes of the charge and current images:a ji v=a ji q.However,a horizontal dipole prob-lem cannot be solved,even approximately,for the same image amplitudes,and requires the different amplitudes for various images.Now,solution of(4)is expressed as follows:a ii q=a ii v=εi−εmεi+εma m i q=a m i v=2εmεi+εm(5a)a ii h=µm−µiµi+µma m i h=2µiµi+µm(5b)C.Derivation of Image Amplitudes A jiktOnce the image amplitudes a ji t of equivalent interface prob-lem of Fig.3(b)and(c)are obtained,a recursive procedureto derive the image amplitudes A jiktof equivalent glass antenna problem of Fig.2(b)can be developed.Describe it for the source current J placed at the distance d over the layer(in the region i=1).To satisfy boundary conditions on the upper dielectric inter-face in Fig.2(b),following the earlier refined MIT,we intro-duce,along with source current J radiating in the source region 1,two image currents situated on equal distances d from the interface:mirror current J−1with amplitude A11−1t=a11t again radiating in the region1and space-like image J0with amplitude A210t=a21t radiating in region2.Now,to satisfy boundary conditions on the bottom interface, we consider the radiation problem for the image current J0 with amplitude a21t in the region2in the presence of bottom interface.This requires two additional image currents situated at equal distances l+d from the bottom interface: J−2with amplitude A21−2t=a21t a22t radiating in the region2and J0with amplitude A310t=a21t a12t radiating in the region3.Next,we should adjust the boundary conditions on the up-per interface disbalanced due to the radiation of image current J−2with amplitude a21t a22t in the region2in the presence of upper interface.This produces a pair of additional image cur-rents situated at equal distances2l+d from the upper interface: mirror image J1with amplitude A211t=a21t(a22t)2radiating inregion2and J−2with amplitude A11−2t=a21t a22t a12t radiating in region1.Further,recursively applying the refined MIT results in the following amplitudes of image currents J k due to the source current J in region i=1:A11−1t=a11t A11−kt=a21t a12t(a22t)2k−3,k=2,3,...(6a) A21−kt=a21t(a22t)2k−3,k=2,3,...(6b) A21kt=a21t(a22t)2k,k=0,1,2,...(6c) A31kt=a21t a12t(a22t)2k,k=0,1,2,....(6d) The similar formulas for the source current J placed inside the layer(i=2)are given in[36].In the next section,these results will be validated by compar-ison with the accurate Sommerfeld solution.D.MoM Solution to the Equivalent Glass Antenna Model The equivalent glass antenna model shown in Fig.2(b)allows to write the equivalent current and charge(i.e.,the divergence of current)associated with antenna element A at any observation region j=1,2,3asJ = Jδij +k[A jikvJ vk+A jikhJ hk](7)i/ω∇ J =i/ω∇ Jδij+kA jikq∇ J k(7a)where J is the original current on element A in the i th region,δij is the Kronecker delta, J k= k J is the current on the k th image, k is the imaging operator, J v k=( J k n) n and J h k= J k− J v k are the vertical and horizontal components of the k th imagecurrents,and n is a unit normal vector to the dielectric interface.The expression(7)can be viewed as J = ( J),where isthe transforming operator.Substituting(7)into(1)along withmodified excitation g = ( g)leads to the following equivalentboundary value problem on the antenna element geometry:L G( J)= g G(8) whereL G=L g G= g(8a) are the modified boundary operator and excitation in the glass area including the dielectric effect,and automatically satisfying the boundary conditions on the dielectric interfaces. Equation(8)allows applying the traditional MoM scheme of Section II to the equivalent model shown in Fig.2(b)with expan-sion functions taken on both the original and image geometries, and testing performed only on original geometry.The expression(8a)allows deriving the hybrid MoM schemewithfinite-sized glass antenna model incorporated into the fullMoM geometry.E.Finite-Sized and Curved Glass Antenna Models Although the equivalent glass antenna model shown in Fig.2(b)is derived for the infinite dielectric layers,it also may be approximately applied tofinite-sized glass antenna struc-tures.Moreover,slightly curved glass antenna geometries maybe handled.For this purpose,afinite-sized dielectric substrate issubdivided into the separateflat areas,and each antenna elementis associated with nearby glass area.The antenna elements nearthis area are considered to radiate,such as in the presence of theinfinite dielectric substrate being extension of this glass area.F.Hybrid MoM SchemeIn order to incorporate the equivalent glass antenna modelinto the full MoM geometry,we divide the full geometry intothe basis,e.g.,vehicle,geometry B,and glass antenna elementgeometry A,and modify the boundary operator and excitationon A,such as to include the dielectric effect.Thereby,we reducethe boundary value problem(1)to the following set:L( J B)+L G( J A)= g B+ g A G on S B(9a)L G( J B)+L G( J A)= g B G+ g A G on S A(9b) where L G and g G are the modified boundary operator and ex-citation defined by(8a),and superscripts B and A stand forthe basis and glass antenna elements,respectively.The set(9)represents the hybrid MoM formulation of the problem to besolved.Next,applying the traditional MoM scheme of Section IIwith expansion functions{ f n( r )}N n=1and weighting functions { w m( r)}N m=1,with N=N A+N B,to(9)leads to the following linear set of algebraic equations written in a block matrix formas[Z B Bm n][Z B Am n][Z ABm n][Z AAm n][I B n][I A n]=[V B Bm]+[V B Am][V ABm]+[V AAm](10)with MoM impedance matrix elements Z B Bm n= w B m,L f B nand Z βαm n= wβm,L G fαn ,and excitation elements V B Bm= w B m, g B ,V βαm= wβm, gαG ,andα,β={A,B}.The linear set(10)incorporates the equivalent glass antenna model into the full MoM geometry,and therefore properly ac-counts for the dielectric effect on glass antenna elements.IV.R ESULTS AND D ISCUSSIONThe suggested theoretical approach has been validated both for the equivalent glass antenna model and the hybrid MoM scheme constructed on this approach.A.Equivalent Glass Antenna ModelThe developed model has been validated on a two-element dipole array printed on the glass surface,as depicted in Fig.4. One dipole of the array is passive and the other active and fed by a lumped voltage applied to the center of the dipole considered as antenna port.The input resistance and reactance at the antenna port are calculated.The dipoles are formed on the metallic strips of width w= 2mm and total length L=900mm.The distance between the dipoles is d=40mm.The dielectric substrate is of the thickness l=6mm and relative permittivityεr=6.6.TheFig.4.Schematic representation of validationsetup.Fig.5.Input impedance of dipole antenna.strips are assumed to be perfectly conducting and the dielectric substrate infinitely extended.Fig.5shows the comparison of the results calculated for the equivalent glass antenna model (MoM code “TriD”[10],included in the program package “EMC Studio”[40]),with those obtained by the accurate Sommerfeld solution,and the equivalent wire approach by Popovi´c [41].The analysis shown in Fig.5shows that results obtained by using the equivalent glass antenna model closely agree with the accurate Sommerfeld solution,whereas those obtained by equiv-alent wire approach reveal the alteration of resonance maxima and their shifting to lower frequencies.Thus,these results vali-date the equivalent glass antenna model and show its advantage compared to the equivalent wire approach.B.Hybrid MoM SchemeThe hybrid MoM scheme was validated on a number of mod-els with glass antennas by comparison of simulated results with those obtained by measurements.1)Complex Grid Glass Antenna:The first validation setup shown in Fig.6has been developed to compare the scatter-ing matrix of a complex grid antenna printed on a dielectric substrate and placed above a metallic plate.Two terminals of antenna are connected to the network analyzer via Bayonet Neill-Concelman (BNC)connectors,the outputs of which are considered as ports.The reflection coefficient at each port and transmission coefficient between the ports are measured.A schematic representation of the measurement setup with di-mensions of included elements is depicted in Fig.7.The metallic strips of antenna are of width w =2mm and length L =510mm.The dielectric substrate is of width W =290mm,height H =800mm,thickness l =6mm,relative permittivity εr =6.6,andFig.6.View of the measurement setup for the complex gridantenna.Fig.7.Schematic representation of the measurement setup.has a loss tangent of tan(δ)=0.02.The metallic plate is of the size 1m ×2m.The simulation model of the measurement setup consists of 405glass antenna triangles to model the glass antenna elements,18wire segments to model the antenna ports,and 1082metal triangles to model the metallic plate,on which the antenna is mounted,giving a total of N =2018unknowns.The metallic elements are taken to be perfectly conducting.In order to model the connectors at the antenna terminals,network transmission line elements of the length 22mm and characteristic impedance 50Ωare used.One of the antenna ports is considered to be active and excited by a lumped voltage with a series resistance of 50Ω.The other port is passive and loaded by the same resistance.Further,the active and passive ports are swapped to simulate the complete scattering matrix.parison of the measurement and simulation results for complex grid antenna.(a)Reflection coefficient.(b)Transmission coefficient.Fig.8shows the comparison between the simulation and mea-surement results,and also the simulations performed without the dielectric(glass).The comparison of simulated results with and without the dielectric shows that neglecting the dielectric leads to the considerable shifting and distortion of resonances,and is thus no longer acceptable starting from the frequencies of 30MHz.The comparison between the simulated and measured results with the glass included shows a rather good agreement in a wide frequency range from30to300MHz.Specifically,these results show a very good prediction of locations and maxima of all resonances.A slight deviation between the compared results in resonance minima and discrepancies in higher frequencies may be caused by some uncertainty of the measurement setup and imperfection of the simulation model.2)Coupling From Active Cable to Grid Glass Antenna:The next validation setup shown in Fig.9has been developed to compare the coupling characteristics of an active single-wire cable to a passive complex grid antenna shown in Fig.7,when both are placed over the metallic ground plate.In one terminal, each of the antenna and the active cable are connected to the network analyzer via the BNC connectors,while the second ter-minals are grounded through50-Ωresistances.The transmission coefficient from the cable to the antenna is measured.A schematic representation of the measurement setup with dimensions of the included elements is depicted in Fig.10.Fig.9.View of measurement setup for the couplingproblem.Fig.10.Schematic representation of the measurement setup.Besides elements shown in Fig.7,this setup includes a single-wire cable of length L w=1.52m,height over plate h=20mm, radius r=0.33mm,dielectric insulation of thickness l d= 0.28mm,and relative permittivityεrd=3.8.The simulation model of the measurement setup consists of 405glass antenna triangles to model the glass antenna elements, 181wire segments to model the active cable and antenna ports, and2328metal triangles to model the metallic plate,giving a total of N=4037unknowns.The metallic elements are taken to be perfectly conducting.The connectors at the active cable and the antenna terminals are modeled by network transmission line elements of length 22mm and characteristic impedance50Ω.The port1on active cable termination is excited by a lumped voltage with a series resistance of50Ω.The port2is passive and loaded by the50-Ωresistance.Fig.11.Transmission coefficient from the cable toantenna.Fig.12.Simulation model of the measurement setup.(a)Full car.(b)Glass antenna.Fig.11shows the comparison between the simulated and measured results for the transmission coefficient |S 21|from the cable to the antenna.As in the previous example,the simulated results agree rather well with measured data up to the frequen-cies of 300MHz.A slight deviation between the results in the resonance minima may be caused,additionally,by inexact in-formation of the parameters of wire insulation.3)Full-Car Model With Integrated Glass Antenna:Finally,a full-car model with a rear window glass antenna has been measured and simulated to compare the scattering matrices of a realistic glass antenna integrated in a car.Fig.12(a)shows a simulation model of the measurement setup,while Fig.12(b)shows a detail of the considered glass antenna with its FM port.The simulation model of the measurement setup consists of 2477glass antenna triangles to model the glass antenna ele-ments,67wire segments to model the wire to body connections and antenna ports,and 19052metal triangles to model the car body shell,giving a total of N =31028unknowns.The metallic elements are taken to be perfectly conducting.The curved glass surface is represented by 5210triangles,which do not contribute to the main geometry.The dielectric substrate is of thickness l =3.14mm,relative permittivity εr =7.5,and has a dielectric loss tangent of tan(δ)=0.02.The port of the antenna is assumed to be at the end of the BNC connectors attached to the antenna terminals.Therefore,these connectors are included as network transmission line elements of length 64mm and a characteristic impedance of 50Ω.parison of the measurement and simulation results for a full-carmodel.Fig.13shows the measured and simulated results for the reflection coefficient |S 11|at the FM antenna port of the glass antenna integrated into the full-car parison between these results shows that,even for very complex models,the simulated results are in a close agreement with measurement data at all frequencies in the range from 30to 300MHz.V .C ONCLUSIONAn equivalent model of the glass antenna structure has shown to be an appropriate model to obtain the reasonable results com-pared to the traditional Green’s function Sommerfeld approach.The hybrid MoM scheme incorporating equivalent glass an-tenna model has shown to be capable to properly describe the combined MoM and finite-sized and curved glass antenna ge-ometries.Specifically,a good agreement between the simulated and measured EMC characteristics for all the measurement se-tups has been shown in a wide frequency range from 30to 300MHz.R EFERENCES[1]T.Zwick,K.Schmitt,and W.Wiesbeck,“Funkkommunikation durchFahrzeugscheiben,”ATZ Automobiltech.Z.,vol.99,pp.220–226,Apr.1997.[2]J.C.Batchelor,ngley,H.Endo,and M.Saito,“Modeling ofvehicles with integrated antennas,”Microw.Opt.Technol.Lett.,vol.27,no.6,pp.404–407,2000.[3]J.C.Batchelor,ngley,and H.Endo,“On-glass mobile antenna per-formance modeling,”in IEE Proc.,Microw.Antennas Propag.,vol.148,no.4,pp.233–238,Aug.2001.[4]R.Abou-Jaoude and E.K.Walton,“Numerical modelling of on-glassconformal automobile antennas,”IEEE Trans.Antennas Propag.,vol.46,no.6,pp.845–852,Jun.1998.[5]L.Low,ngley,and J.Batchelor,“Modelling and performance ofconformal automotive antennas,”IET Microw.Antennas Propag.,vol.1,no.5,pp.973–979,2007.[6] A.R.Ruddle,H.Zhang,L.Low,J.Rigelsford,and ngley,“Numeri-cal investigation of the impact of dielectric components on electromagnetic field distributions in the passenger compartment of a vehicle,”in Proc.20th Int.Zurich Symp.EMC ,Zurich,Switzerland,2009,pp.213–216.[7]R.F.Harrington and J.R.Mautz,“An impedance sheet approximationfor thin dielectric shells,”IEEE Trans.Antennas Propag.,vol.23,no.4,pp.531–534,Jul.1975.[8] E.H.Newman,“A user’s manual for electromagnetic surface patch code:Version V (ESP5),”b.,Ohio State Univ.,Columbus,OH,Oct.1998.。
薛定谔—麦克斯韦尔方程径向解的存在性和多重性(英文)

In 1887, the German physicist Erwin Schrödinger proposed a radial solution to the Maxwell-Schrödinger equation. This equation describes the behavior of an electron in an atom and is used to calculate its energy levels. The radial solution was found to be valid for all values of angular momentum quantum number l, which means that it can describe any type of atomic orbital.The existence and multiplicity of this radial solution has been studied extensively since then. It has been shown that there are infinitely many solutions for each value of l, with each one corresponding to a different energy level. Furthermore, these solutions can be divided into two categories: bound states and scattering states. Bound states have negative energies and correspond to electrons that are trapped within the atom; scattering states have positive energies and correspond to electrons that escape from the atom after being excited by external radiation or collisions with other particles.The existence and multiplicity of these solutions is important because they provide insight into how atoms interact with their environment through electromagnetic radiation or collisions with other particles. They also help us understand why certain elements form molecules when combined together, as well as why some elements remain stable while others decay over time due to radioactive processes such as alpha decay or beta decay.。
XPSPEAK 说明书

Using XPSPEAK Version 4.1 November 2000Contents Page Number XPS Peak Fitting Program for WIN95/98 XPSPEAK Version 4.1 (1)Program Installation (1)Introduction (1)First Version (1)Version 2.0 (1)Version 3.0 (1)Version 3.1 (2)Version 4.0 (2)Version 4.1 (2)Future Versions (2)General Information (from R. Kwok) (3)Using XPS Peak (3)Overview of Processing (3)Appearance (4)Opening Files (4)Opening a Kratos (*.des) text file (4)Opening Multiple Kratos (*.des) text files (5)Saving Files (6)Region Parameters (6)Loading Region Parameters (6)Saving Parameters (6)Available Backgrounds (6)Averaging (7)Shirley + Linear Background (7)Tougaard (8)Adding/Adjusting the Background (8)Adding/Adjusting Peaks (9)Peak Types: p, d and f (10)Peak Constraints (11)Peak Parameters (11)Peak Function (12)Region Shift (13)Optimisation (14)Print/Export (15)Export (15)Program Options (15)Compatibility (16)File I/O (16)Limitations (17)Cautions for Peak Fitting (17)Sample Files: (17)gaas.xps (17)Cu2p_bg.xps (18)Kratos.des (18)ASCII.prn (18)Other Files (18)XPS Peak Fitting Program for WIN95/98 XPSPEAKVersion 4.1Program InstallationXPS Peak is freeware. Please ask RCSMS lab staff for a copy of the zipped 3.3MB file, if you would like your own copyUnzip the XPSPEA4.ZIP file and run Setup.exe in Win 95 or Win 98.Note: I haven’t successfully installed XPSPEAK on Win 95 machines unless they have been running Windows 95c – CMH.IntroductionRaymond Kwok, the author of XPSPEAK had spent >1000 hours on XPS peak fitting when he was a graduate student. During that time, he dreamed of many features in the XPS peak fitting software that could help obtain more information from the XPS peaks and reduce processing time.Most of the information in this users guide has come directly from the readme.doc file, automatically installed with XPSPEAK4.1First VersionIn 1994, Dr Kwok wrote a program that converted the Kratos XPS spectral files to ASCII data. Once this program was finished, he found that the program could be easily converted to a peak fitting program. Then he added the dreamed features into the program, e.g.∙ A better way to locate a point at a noise baseline for the Shirley background calculations∙Combine the two peaks of 2p3/2 and 2p1/2∙Fit different XPS regions at the same timeVersion 2.0After the first version and Version 2.0, many people emailed Dr Kwok and gave additional suggestions. He also found other features that could be put into the program.Version 3.0The major change in Version 3.0 is the addition of Newton’s Method for optimisation∙Newton’s method can greatly reduce the optimisation time for multiple region peak fitting.Version 3.11. Removed all the run-time errors that were reported2. A Shirley + Linear background was added3. The Export to Clipboard function was added as requested by a user∙Some other minor graphical features were addedVersion 4.0Added:1. The asymmetrical peak function. See note below2. Three additional file formats for importing data∙ A few minor adjustmentsThe addition of the Asymmetrical Peak Function required the peak function to be changed from the Gaussian-Lorentzian product function to the Gaussian-Lorentzian sum function. Calculation of the asymmetrical function using the Gaussian-Lorentzian product function was too difficult to implement. The software of some instruments uses the sum function, while others use the product function, so both functions are available in XPSPEAK.See Peak Function, (Page 12) for details of how to set this up.Note:If the selection is the sum function, when the user opens a *.xps file that was optimised using the Gaussian-Lorentzian product function, you have to re-optimise the spectra using the Gaussian-Lorentzian sum function with a different %Gaussian-Lorentzian value.Version 4.1Version 4.1 has only two changes.1. In version 4.0, the printed characters were inverted, a problem that wasdue to Visual Basic. After about half year, a patch was received from Microsoft, and the problem was solved by simply recompiling the program2. The import of multiple region VAMAS file format was addedFuture VersionsThe author believes the program has some weakness in the background subtraction routines. Extensive literature examination will be required in order to revise them. Dr Kwok intends to do that for the next version.General Information (from R. Kwok)This version of the program was written in Visual Basic 6.0 and uses 32 bit processes. This is freeware. You may ask for the source program if you really want to. I hope this program will be useful for people without modern XPS software. I also hope that the new features in this program can be adopted by the XPS manufacturers in the later versions of their software.If you have any questions/suggestions, please send an email to me.Raymund W.M. KwokDepartment of ChemistryThe Chinese University of Hong KongShatin, Hong KongTel: (852)-2609-6261Fax:(852)-2603-5057email: rmkwok@.hkI would like to thank the comments and suggestions from many people. For the completion of Version 4.0, I would like to think Dr. Bernard J. Flinn for the routine of reading Leybold ascii format, Prof. Igor Bello and Kelvin Dickinson for providing me the VAMAS files VG systems, and my graduate students for testing the program. I hope I will add other features into the program in the near future.R Kwok.Using XPS PeakOverview of Processing1. Open Required Files∙See Opening Files (Page 4)2. Make sure background is there/suitable∙See Adding/Adjusting the Background, (Page 8)3. Add/adjust peaks as necessary∙See Adding/Adjusting Peaks, (Page 9), and Peak Parameters, (Page 11)4. Save file∙See Saving Files, (Page 6)5. Export if necessary∙See Print/Export, (Page 15)AppearanceXPSPEAK opens with two windows, one above the other, which look like this:∙The top window opens and displays the active scan, adds or adjusts a background, adds peaks, and loads and saves parameters.∙The lower window allows peak processing and re-opening and saving dataOpening FilesOpening a Kratos (*.des) text file1. Make sure your data files have been converted to text files. See the backof the Vision Software manual for details of how to do this. Remember, from the original experiment files, each region of each file will now be a separate file.2. From the Data menu of the upper window, choose Import (Kratos)∙Choose directory∙Double click on the file of interest∙The spectra open with all previous processing INCLUDEDOpening Multiple Kratos (*.des) text files∙You can open up a maximum of 10 files together.1. Open the first file as above∙Opens in the first region (1)2. In the XPS Peak Processing (lower) window, left click on 2(secondregion), which makes this region active3. Open the second file as in Step2, Opening a Kratos (*.des) text file,(Page 4)∙Opens in the second region (2)∙You can only have one description for all the files that are open. Edit with a click in the Description box4. Open further files by clicking on the next available region number thenfollowing the above step.∙You can only have one description for all the files that are open. Edit with a click in the Description boxDescriptionBox 2∙To open a file that has already been processed and saved using XPSPEAK, click on the Open XPS button in the lower window. Choose directory and file as normal∙The program can store all the peak information into a *.XPS file for later use. See below.Saving Files1. To save a file click on the Save XPS button in the lower window2. Choose Directory3. Type in a suitable file name4. Click OK∙Everything that is open will be saved in this file∙The program can also store/read the peak parameter files (*.RPA)so that you do not need to re-type all the parameters again for a similar spectrum.Region ParametersRegion Parameters are the boundaries or limits you have used to set up the background and peaks for your files. These values can be saved as a file of the type *.rpa.Note that these Region Parameters are completely different from the mathematical parameters described in Peak Parameters, (Page 11) Loading Region Parameters1. From the Parameters menu in the upper window, click on Load RegionParameters2. Choose directory and file name3. Click on Open buttonSaving Parameters1. From the Parameters menu in the XPS Peak Fit (Upper) window, clickon Save Region Parameters2. Choose directory and file name3. Click on the Save buttonAvailable BackgroundsThis program provides the background choices of∙Shirley∙Linear∙TougaardAveraging∙ Averaging at the end points of the background can reduce the time tofind a point at the middle of a noisy baseline∙ The program includes the choices of None (1 point), 3, 5, 7, and 9point average∙ This will average the intensities around the binding energy youselect.Shirley + Linear Background1. The Shirley + Linear background has been added for slopingbackgrounds∙ The "Shirley + Linear" background is the Shirley background plus astraight line with starting point at the low BE end-point and with a slope value∙ If the slope value is zero , the original Shirley calculation is used∙ If the slope value is positive , the straight line has higher values atthe high BE side, which can be used for spectra with higher background intensities at the high BE side∙ Similarly, a negative slope value can be used for a spectrum withlower background intensities at the high BE side2. The Optimization button may be used when the Shirley background is higher at some point than the signal intensities∙ The program will increase the slope value until the Shirleybackground is below the signal intensities∙ Please see the example below - Cu2p_bg.xps - which showsbackground subtraction using the Shirley method (This spectrum was sent to Dr Kwok by Dr. Roland Schlesinger).∙ A shows the problematic background when the Shirley backgroundis higher than the signal intensities. In the Shirley calculation routine, some negative values were generated and resulted in a non-monotonic increase background∙ B shows a "Shirley + Linear" background. The slope value was inputby trial-and-error until the background was lower than the signal intensities∙ C was obtained using the optimisation routineA slope = 0B slope = 11C slope = 15.17Note: The background subtraction calculation cannot completely remove the background signals. For quantitative studies, the best procedure is "consistency". See Future Versions, (Page 2).TougaardFor a Tougaard background, the program can optimise the B1 parameter by minimising the "square of the difference" of the intensities of ten data points in the high binding energy side of the range with the intensities of the calculated background.Adding/Adjusting the BackgroundNote: The Background MUST be correct before Peaks can be added. As with all backgrounds, the range needs to include as much of your peak as possible and as little of anything else as possible.1. Make sure the file of interest is open and the appropriate region is active2. Click on Background in the upper window∙The Region 0 box comes up, which contains the information about the background3. Adjust the following as necessary. See Note.∙High BE (This value needs to be within the range of your data) ∙Low BE (This value needs to be within the range of your data) NOTE: High and Low BE are not automatically within the range of your data. CHECK CAREFULLY THAT BOTH ENDS OF THE BACKGROUND ARE INSIDE THE EDGE OF YOUR DATA. Nothing will happen otherwise.∙No. of Ave. Pts at end-points. See Averaging, (Page 7)∙Background Type∙Note for Shirley + Linear:To perform the Shirley + Linear Optimisation routine:a) Have the file of interest openb) From the upper window, click on Backgroundc) In the resulting box, change or optimise the Shirley + LinearSlope as desired∙Using Optimize in the Shirley + Linear window can cause problems. Adjust manually if necessary3. Click on Accept when satisfiedAdding/Adjusting PeaksNote: The Background MUST be correct before peaks can be added. Nothing will happen otherwise. See previous section.∙To add a peak, from the Region Window, click on Add Peak ∙The peak window appears∙This may be adjusted as below using the Peak Window which will have opened automaticallyIn the XPS Peak Processing (lower) window, there will be a list of Regions, which are all the open files, and beside each of these will be numbers representing the synthetic peaks included in that region.Regions(files)SyntheticPeaks1. Click on a region number to activate that region∙The active region will be displayed in the upper window2. Click on a peak number to start adjusting the parameters for that peak.∙The Processing window for that peak will open3. Click off Fix to adjust the following using the maximum/minimum arrowkeys provided:∙Peak Type. (i.e. orbital – s, p, d, f)∙S.O.S (Δ eV between the two halves of the peak)∙Position∙FWHM∙Area∙%Lorenzian-Gaussian∙See the notes for explanations of how Asymmetry works.4. Click on Accept when satisfiedPeak Types: p, d and f.1. Each of these peaks combines the two splitting peaks2. The FWHM is the same for both the splitting peaks, e.g. a p-type peakwith FWHM=0.7eV is the combination of a p3/2 with FWHM at 0.7eV anda p1/2 with FWHM at 0.7eV, and with an area ratio of 2 to 13. If the theoretical area ratio is not true for the split peaks, the old way ofsetting two s-type peaks and adding the constraints should be used.∙The S.O.S. stands for spin orbital splitting.Note: The FWHM of the p, d or f peaks are the FWHM of the p3/2,d5/2 or f7/2, respectively. The FWHM of the combined peaks (e.g. combination of p3/2and p1/2) is shown in the actual FWHM in the Peak Parameter Window.Peak Constraints1. Each parameter can be referenced to the same type of parameter inother peaks. For example, for four peaks (Peak #0, 1, 2 and 3) with known relative peak positions (0.5eV between adjacent peaks), the following can be used∙Position: Peak 1 = Peak 0 + 0.5eV∙Position: Peak 2 = Peak 1 + 0.5eV∙Position: Peak 3 = Peak 2 + 0.5eV2. You may reference to any peak except with looped references.3. The optimisation of the %GL value is allowed in this program.∙ A suggestion to use this feature is to find a nice peak for a certain setting of your instrument and optimise the %GL for this peak.∙Fix the %GL in the later peak fitting process when the same instrument settings were used.4. This version also includes the setting of the upper and lower bounds foreach parameter.Peak ParametersThis program uses the following asymmetric Gaussian-Lorentzian sumThe program also uses the following symmetrical Gaussian-Lorentzian product functionPeak FunctionNote:If the selection is the sum function, when the user opens a *.xps file that was optimised using the Gaussian-Lorentzian product function, you have to re-optimise the spectra using the Gaussian-Lorentzian sum function with a different %Gaussian-Lorentzian value.∙You can choose the function type you want1. From the lower window, click on the Options button∙The peak parameters box comes up∙Select GL sum for the Gaussian-Lorentzian sum function∙Select GL product for the Gaussian-Lorentzian product function. 2. For the Gaussian-Lorentzian sum function, each peak can have sixparameters∙Peak Position∙Area∙FWHM∙%Gaussian-Lorentzian∙TS∙TLIf anyone knows what TS or TL might be, please let me know. Thanks, CMH3. Each peak in the Gaussian-Lorentzian product function can have fourparameters∙Peak Position∙Area∙FWHM∙%Gaussian-LorentzianSince peak area relates to the atomic concentration directly, we use it as a peak parameter and the peak height will not be shown to the user.Note:For asymmetric peaks, the FWHM only refers to the half of the peak that is symmetrical. The actual FWHM of the peak is calculated numerically and is shown after the actual FWHM in the Peak Parameter Window. If the asymmetric peak is a doublet (p, d or f type peak), the actual FWHM is the FWHM of the doublet.Region ShiftA Region Shift parameter was added under the Parameters menu∙Use this parameter to compensate for the charging effect, the fermi level shift or any change in the system work function∙This value will be added to all the peak positions in the region for fitting purposes.An example:∙ A polymer surface is positively charged and all the peaks are shifted to the high binding energy by +0.5eV, e.g. aliphatic carbon at 285.0eV shifts to 285.5eV∙When the Region Shift parameter is set to +0.5eV, 0.5eV will be added to all the peak positions in the region during peak fitting, but the listed peak positions are not changed, e.g. 285.0eV for aliphatic carbon. Note: I have tried this without any actual shift taking place. If someone finds out how to perform this operation, please let me know. Thanks, CMH.In the meantime, I suggest you do the shift before converting your files from the Vision Software format.OptimisationYou can optimise:1. A single peak parameter∙Use the Optimize button beside the parameter in the Peak Fitting window2. The peak (the peak position, area, FWHM, and the %GL if the "fix" box isnot ticked)∙Use the Optimize Peak button at the base of the Peak Fitting window3. A single region (all the parameters of all the peaks in that region if the"fix" box is not ticked)∙Use the Optimize Region menu (button) in the upper window4. All the regions∙Use the Optimize All button in the lower window∙During any type of optimisation, you can press the "Stop Fitting" button and the program will stop the process in the next cycle.Print/ExportIn the XPS Peak Fit or Region window, From the Data menu, choose Export or Print options as desiredExport∙The program can export the ASCII file of spectrum (*.DAT) for making high quality figures using other software (e.g. SigmaPlot)∙It can export the parameters (*.PAR) for further calculations (e.g. use Excel for atomic ratio calculations)∙It can also copy the spectral image to the system clipboard so that the spectral image can be pasted into a document (e.g. MS WORD). Program Options1. The %tolerance allows the optimisation routine to stop if the change inthe difference after one loop is less that the %tolerance2. The default setting of the optimisation is Newton's method∙This method requires a delta value for the optimisation calculations ∙You may need to change the value in some cases, but the existing setting is enough for most data.3. For the binary search method, it searches the best fit for each parameterin up to four levels of value ranges∙For example, for a peak position, in first level, it calculates the chi^2 when the peak position is changed by +2eV, +1.5eV, +1eV, +0.5eV,-0.5eV, -1eV, -1.5eV, and -2eV (range 2eV, step 0.5eV) ∙Then, it selects the position value that gives the lowest chi^2∙In the second level, it searches the best values in the range +0.4eV, +0.3eV, +0.2eV, +0.1eV, -0.1eV, -0.2eV, -0.3eV, and -0.4eV (range0.4eV, step 0.1eV)∙In the third level, it selects the best value in +0.09eV, +0.08eV, ...+0.01eV, -0.01eV, ...-0.09eV∙This will give the best value with two digits after decimal∙Level 4 is not used in the default setting∙The range setting and the number of levels in the option window can be changed if needed.4. The Newton's Method or Binary Search Method can be selected byclicking the "use" selection box of that method.5. The selection of the peak function is also in the Options window.6. The user can save/read the option parameters with the file extension*.opa∙The program reads the default.opa file at start up. Therefore, the user can customize the program options by saving the selectionsinto the default.opa file.CompatibilityThe program can read:∙Kratos text (*.des) files together with the peak fitting parameters in the file∙The ASCII files exported from Phi's Multiplex software∙The ASCII files of Leybold's software∙The VAMAS file format∙For the Phi, Leybold and VAMAS formats, multiple regions can be read∙For the Phi format, if the description contains a comma ",", the program will give an error. (If you get the error, you may use any texteditor to remove the comma)The program can also import ASCII files in the following format:Binding Energy Value 1 Intensity Value 1Binding Energy Value 2 Intensity Value 2etc etc∙The B.E. list must be in ascending or descending order, and the separation of adjacent B.E.s must be the same∙The file cannot have other lines before and after the data∙Sometimes, TAB may cause a reading error.File I/OThe file format of XPSPEAK 4.1 is different from XPSPEAK 3.1, 3.0 and 2.0 ∙XPSPEAK 4.1 can read the file format of XPSPEAK 3.1, 3.0 and 2.0, but not the reverse∙File format of 4.1 is the same as that of 4.0.LimitationsThis program limits the:∙Maximum number of points for each spectrum to 5000∙Maximum of peaks for all the regions to 51∙For each region, the maximum number of peaks is 10. Cautions for Peak FittingSome graduate students believe that the fitting parameters for the best fitted spectrum is the "final answer". This is definitely not true. Adding enough peaks can always fit a spectrum∙Peak fitting only assists the verification of a model∙The user must have a model in mind before adding peaks to the spectrum!Sample Files:gaas.xpsThis file contains 10 spectra1. Use Open XPS to retrieve the file. It includes ten regions∙1-4 for Ga 3d∙5-8 for Ga 3d∙9-10 for S 2p2. For the Ga 3d and As 3d, the peaks are d-type with s.o.s. = 0.3 and 0.9respectively3. Regions 4 and 8 are the sample just after S-treatment4. Other regions are after annealing5. Peak width of Ga 3d and As 3d are constrained to those in regions 1 and56. The fermi level shift of each region was determined using the As 3d5/2peak and the value was put into the "Region Shift" of each region7. Since the region shift takes into account the Fermi level shift, the peakpositions can be easily referenced for the same chemical components in different regions, i.e.∙Peak#1, 3, 5 of Ga 3d are set equal to Peak#0∙Peak#8, 9, 10 of As 3d are set equal to Peak#78. Note that the %GL value of the peaks is 27% using the GL sum functionin Version 4.0, while it is 80% using the GL product function in previous versions.18 Cu2p_bg.xpsThis spectrum was sent to me by Dr. Roland Schlesinger. It shows a background subtraction using the Shirley + Linear method∙See Shirley + Linear Background, (Page 7)Kratos.des∙This file shows a Kratos *.des file∙This is the format your files should be in if they have come from the Kratos instrument∙Use import Kratos to retrieve the file. See Opening Files, (Page 4)∙Note that the four peaks are all s-type∙You may delete peak 2, 4 and change the peak 1,3 to d-type with s.o.s. = 0.7. You may also read in the parameter file: as3d.rpa. ASCII.prn∙This shows an ASCII file∙Use import ASCII to retrieve the file∙It is a As 3d spectrum of GaAs∙In order to fit the spectrum, you need to first add the background and then add two d-type peaks with s.o.s.=0.7∙You may also read in the parameter file: as3d.rpa.Other Files(We don’t have an instrument that produces these files at Auckland University., but you may wish to look at them anyway. See the readme.doc file for more info.)1. Phi.asc2. Leybold.asc3. VAMAS.txt4. VAMASmult.txtHave Fun! July 1, 1999.。
国家电子NI 9227 四通道、五臂、24位、同时、渠道间隔离模拟输入模块操作指南和规格说明书
Caution Ensure that hazardous voltage wiring is performed only by qualified personnel adhering to local electrical standards. Caution Do not mix hazardous voltage circuits and human-accessible circuits on the same module. Caution Make sure that devices and circuits connected to the module are properly insulated from human contact.
Services /services
2 | | NI 9227 Operating Instructions and Specifications
Safety Guidelines
Operate the NI 9227 only as described in these operating instructions.
Figure 5. Input Circuitry for One Channel of the NI 9227
AI+C
Amplifier Prefilter
NI 9227
NI 9227 Operating Instructions and Specifications | © National Instruments | 9
Related Information
lyapunov指数与光滑定理

Lyapunov指数和光滑定理是两个不同的概念,分别用于描述动力系统和微分方程的不同性质。
1. Lyapunov指数:Lyapunov指数是一种描述相空间中相邻轨迹平均指数发散率的数值特征。
它被用于识别混沌运动,是混沌理论中的重要概念。
当Lyapunov指数大于0时,系统运动会进入混沌状态;当Lyapunov指数小于0时,系统的运动状态会趋于稳定;当Lyapunov 指数等于0时,系统则处于稳定状态。
因此,Lyapunov指数是判断系统是否混沌的重要依据。
2. 光滑定理:光滑定理通常用于微分方程或动力系统的研究中,它描述了系统在某些条件下保持某种性质的稳定性或光滑性的性质。
例如,对于常微分方程或偏微分方程,如果初值问题存在唯一解,并且该解对初值具有连续依赖性,则称该系统满足光滑定理。
综上,Lyapunov指数用于描述动力系统的混沌性质,而光滑定理则用于描述微分方程解的连续依赖性和稳定性。
两者在数学和物理领域中都有广泛的应用。
Entropy changes in the clustering of galaxies in a

Vol.3, No.1, 65-68 (2011)doi:10.4236/ns.2011.31009Natural ScienceEntropy changes in the clustering of galaxies in an expanding universeNaseer Iqbal1,2*, Mohammad Shafi Khan1, Tabasum Masood11Department of Physics, University of Kashmir, Srinagar, India; *Corresponding Author:2Interuniversity Centre for Astronomy and Astrophysics, Pune, India.Received 19 October 2010; revised 23 November 2010; accepted 26 November 2010.ABSTRACTIn the present work the approach-thermody- namics and statistical mechanics of gravitating systems is applied to study the entropy change in gravitational clustering of galaxies in an ex-panding universe. We derive analytically the expressions for gravitational entropy in terms of temperature T and average density n of the par-ticles (galaxies) in the given phase space cell. It is found that during the initial stage of cluster-ing of galaxies, the entropy decreases and fi-nally seems to be increasing when the system attains virial equilibrium. The entropy changes are studied for different range of measuring correlation parameter b. We attempt to provide a clearer account of this phenomena. The entropy results for a system consisting of extended mass (non-point mass) particles show a similar behaviour with that of point mass particles clustering gravitationally in an expanding uni-verse.Keywords:Gravitational Clustering; Thermodynamics; Entropy; Cosmology1. INTRODUCTIONGalaxy groups and clusters are the largest known gravitationally bound objects to have arisen thus far in the process of cosmic structure formation [1]. They form the densest part of the large scale structure of the uni-verse. In models for the gravitational formation of struc-ture with cold dark matter, the smallest structures col-lapse first and eventually build the largest structures; clusters of galaxies are then formed relatively. The clus-ters themselves are often associated with larger groups called super-clusters. Clusters of galaxies are the most recent and most massive objects to have arisen in the hiearchical structure formation of the universe and the study of clusters tells one about the way galaxies form and evolve. The average density n and the temperature T of a gravitating system discuss some thermal history of cluster formation. For a better larger understanding of this thermal history it is important to study the entropy change resulting during the clustering phenomena be-cause the entropy is the quantity most directly changed by increasing or decreasing thermal energy of intraclus-ter gas. The purpose of the present paper is to show how entropy of the universe changes with time in a system of galaxies clustering under the influence of gravitational interaction.Entropy is a measure of how disorganised a system is. It forms an important part of second law of thermody-namics [2,3]. The concept of entropy is generally not well understood. For erupting stars, colloiding galaxies, collapsing black holes - the cosmos is a surprisingly or-derly place. Supermassive black holes, dark matter and stars are some of the contributors to the overall entropy of the universe. The microscopic explanation of entropy has been challenged both from the experimental and theoretical point of view [11,12]. Entropy is a mathe-matical formula. Standard calculations have shown that the entropy of our universe is dominated by black holes, whose entropy is of the order of their area in planck units [13]. An analysis by Chas Egan of the Australian National University in Canberra indicates that the col-lective entropy of all the supermassive black holes at the centers of galaxies is about 100 times higher than previ-ously calculated. Statistical entropy is logrithmic of the number of microstates consistent with the observed macroscopic properties of a system hence a measure of uncertainty about its precise state. Statistical mechanics explains entropy as the amount of uncertainty which remains about a system after its observable macroscopic properties have been taken into account. For a given set of macroscopic quantities like temperature and volume, the entropy is a function of the probability that the sys-tem is in various quantumn states. The more states avail-able to the system with higher probability, the greater theAll Rights Reserved.N. Iqbal et al. / Natural Science 3 (2011) 65-6866 disorder and thus greater the entropy [2]. In real experi-ments, it is quite difficult to measure the entropy of a system. The technique for doing so is based on the thermodynamic definition of entropy. We discuss the applicability of statistical mechanics and thermodynam-ics for gravitating systems and explain in what sense the entropy change S – S 0 shows a changing behaviour with respect to the measuring correlation parameter b = 0 – 1.2. THERMODYNAMIC DESCRIPTION OF GALAXY CLUSTERSA system of many point particles which interacts by Newtonian gravity is always unstable. The basic insta-bilities which may occur involve the overall contraction (or expansion) of the system, and the formation of clus-ters within the system. The rates and forms of these in-stabilities are governed by the distribution of kinetic and potential energy and the momentum among the particles. For example, a finite spherical system which approxi-mately satisfies the viral theorem, contracts slowlycompared to the crossing time ~ ()12G ρ- due to the evaporation of high energy particles [3] and the lack of equipartition among particles of different masses [4]. We consider here a thermodynamic description for the sys-tem (universe). The universe is considered to be an infi-nite gas in which each gas molecule is treated to be agalaxy. The gravitational force is a binary interaction and as a result a number of particles cluster together. We use the same approximation of binary interaction for our universe (system) consisting of large number of galaxies clustering together under the influence of gravitational force. It is important to mention here that the characteri-zation of this clustering is a problem of current interest. The physical validity of the application of thermody-namics in the clustering of galaxies and galaxy clusters has been discussed on the basis of N-body computer simulation results [5]. Equations of state for internal energy U and pressure P are of the form [6]:(3122NTU =-)b (1) (1NTP V=-)b (2) b defines the measuring correlation parameter and is dimensionless, given by [8]()202,23W nb Gm n T r K Tτξ∞=-=⎰,rdr (3)W is the potential energy and K the kinetic energy ofthe particles in a system. n N V = is the average num-ber density of the system of particles each of mass m, T is the temperature, V the volume, G is the universalgravitational constant. (),,n T r ξ is the two particle correlation function and r is the inter-particle distance. An overall study of (),n T r ξ has already been dis-cussed by [7]. For an ideal gas behaviour b = 0 and for non-ideal gas system b varies between 0 and 1. Previ-ously some workers [7,8] have derived b in the form of:331nT b nT ββ--=+ (4) Eq.4 indicates that b has a specific dependence on the combination 3nT -.3. ENTROPY CALCULATIONSThermodynamics and statistical mechanics have been found to be equal tools in describing entropy of a system. Thermodynamic entropy is a non-conserved state func-tion that is of great importance in science. Historically the concept of entropy evolved in order to explain why some processes are spontaneous and others are not; sys-tems tend to progress in the direction of increasing en-tropy [9]. Following statistical mechanics and the work carried out by [10], the grand canonical partition func-tion is given by()3213212,1!N N N N mkT Z T V V nT N πβ--⎛⎫⎡=+ ⎪⎣Λ⎝⎭⎤⎦(5)where N! is due to the distinguishability of particles. Λrepresents the volume of a phase space cell. N is the number of paricles (galaxies) with point mass approxi-mation. The Helmholtz free energy is given by:ln N A T Z =- (6)Thermodynamic description of entropy can be calcu-lated as:,N VA S T ∂⎛⎫=- ⎪∂⎝⎭ (7)The use of Eq.5 and Eq.6 in Eq.7 gives()3120ln ln 13S S n T b b -⎛⎫-=-- ⎪ ⎪⎝⎭- (8) where S 0 is an arbitary constant. From Eq.4 we write()31bn b T β-=- (9)Using Eq.9, Eq.8 becomes as3203ln S S b bT ⎡⎤-=-+⎢⎣⎦⎥ (10)Again from Eq.4All Rights Reserved.N. Iqbal et al. / Natural Science 3 (2011) 65-68 6767()13221n b T b β-⎡⎤=⎢⎣⎦⎥ (11)with the help of Eq.11, Eq.10 becomes as()011ln ln 1322S S n b b b ⎡-=-+-+⎡⎤⎣⎦⎢⎥⎣⎦⎤ (12) This is the expression for entropy of a system consist-ing of point mass particles, but actually galaxies have extended structures, therefore the point mass concept is only an approximation. For extended mass structures we make use of softening parameter ε whose value is taken between 0.01 and 0.05 (in the units of total radius). Following the same procedure, Eq.8 becomes as()320ln ln 13N S S N T N b Nb V εε⎡⎤-=---⎢⎥⎣⎦(13)For extended structures of galaxies, Eq.4 gets modi-fied to()()331nT R b nT R εβαεβαε--=+ (14)where α is a constant, R is the radius of a cell in a phase space in which number of particles (galaxies) is N and volume is V . The relation between b and b ε is given by: ()11b b b εαα=+- (15) b ε represents the correlation energy for extended mass particles clustering gravitationally in an expanding uni-verse. The above Eq.10 and Eq.12 take the form respec-tively as;()()3203ln 111bT b S S b b ααα⎡⎤⎢⎥-=-+⎢⎥+-+-⎢⎥⎣⎦1 (16) ()()()120113ln ln 2111b b b S S n b b ααα⎡⎤-⎡⎤⎢⎥⎣⎦-=-++⎢⎥+-+-⎢⎥⎣⎦1 (17)where2R R εεεα⎛⎫⎛⎫=⎪ ⎪⎝⎭⎝⎭(18)If ε = 0, α = 1 the entropy equations for extended mass galaxies are exactly same with that of a system of point mass galaxies approximation. Eq.10, Eq.12, Eq.16and Eq.17 are used here to study the entropy changes inthe cosmological many body problem. Various entropy change results S – S 0 for both the point mass approxima-tion and of extended mass approximation of particles (galaxies) are shown in (Figures 1and2). The resultshave been calculated analytically for different values ofFigure 1. (Color online) Comparison of isothermal entropy changes for non-point and point mass particles (galaxies) for an infinite gravitating system as a function of average relative temperature T and the parameter b . For non-point mass ε = 0.03 and R = 0.06 (left panel), ε = 0.04 and R = 0.04 (right panel).All Rights Reserved.N. Iqbal et al. / Natural Science 3 (2011) 65-68 68Figure 2. (Color online) Comparison of equi-density entropy changes for non-point and point mass particles (galaxies) for an infinite gravitating system as a function of average relative density n and the parameter b. For non-point mass ε= 0.03 and R = 0.04.R (cell size) corresponding to different values of soften-ing parameter ε. We study the variations of entropy changes S – S0with the changing parameter b for differ-ent values of n and T. Some graphical variations for S – S0with b for different values of n = 0, 1, 100 and aver-age temperature T = 1, 10 and 100 and by fixing value of cell size R = 0.04 and 0.06 are shown. The graphical analysis can be repeated for different values of R and by fixing values of εfor different sets like 0.04 and 0.05. From both the figures shown in 1 and 2, the dashed line represents variation for point mass particles and the solid line represents variation for extended (non-point mass) particles (galaxies) clustering together. It has been ob-served that the nature of the variation remains more or less same except with some minor difference.4. RESULTSThe formula for entropy calculated in this paper has provided a convenient way to study the entropy changes in gravitational galaxy clusters in an expanding universe. Gravity changes things that we have witnessed in this research. Clustering of galaxies in an expanding universe, which is like that of a self gravitating gas increases the gases volume which increases the entropy, but it also increases the potential energy and thus decreases the kinetic energy as particles must work against the attrac-tive gravitational field. So we expect expanding gases to cool down, and therefore there is a probability that the entropy has to decrease which gets confirmed from our theoretical calculations as shown in Figures 1 and 2. Entropy has remained an important contributor to our understanding in cosmology. Everything from gravita-tional clustering to supernova are contributors to entropy budget of the universe. A new calculation and study of entropy results given by Eqs.10, 12, 16 and 17 shows that the entropy of the universe decreases first with the clustering rate of the particles and then gradually in-creases as the system attains viral equilibrium. The gravitational entropy in this paper furthermore suggests that the universe is different than scientists had thought.5. ACKNOWLEDGEMENTSWe are thankful to Interuniversity centre for Astronomy and Astro-physics Pune India for providing a warm hospitality and facilities during the course of this work.REFERENCES[1]Voit, G.M. (2005) Tracing cosmic evolution with clus-ters of galaxies. Reviews of Modern Physics, 77, 207- 248.[2]Rief, F. (1965)Fundamentals of statistical and thermalphysics. McGraw-Hill, Tokyo.[3]Spitzer, L. and Saslaw, W.C. (1966) On the evolution ofgalactic nuclei. Astrophysical Journal, 143, 400-420.doi:10.1086/148523[4]Saslaw, W.C. and De Youngs, D.S. (1971) On the equi-partition in galactic nuclei and gravitating systems. As-trophysical Journal, 170, 423-429.doi:10.1086/151229[5]Itoh, M., Inagaki, S. and Saslaw, W.C. (1993) Gravita-tional clustering of galaxies. Astrophysical Journal, 403,476-496.doi:10.1086/172219[6]Hill, T.L. (1956) Statistical mechanics: Principles andstatistical applications. McGraw-Hill, New York.[7]Iqbal, N., Ahmad, F. and Khan, M.S. (2006) Gravita-tional clustering of galaxies in an expanding universe.Journal of Astronomy and Astrophysics, 27, 373-379.doi:10.1007/BF02709363[8]Saslaw, W.C. and Hamilton, A.J.S. (1984) Thermody-namics and galaxy clustering. Astrophysical Journal, 276, 13-25.doi:10.1086/161589[9]Mcquarrie, D.A. and Simon, J.D. (1997) Physical chem-istry: A molecular approach. University Science Books,Sausalito.[10]Ahmad, F, Saslaw, W.C. and Bhat, N.I. (2002) Statisticalmechanics of cosmological many body problem. Astro-physical Journal, 571, 576-584.doi:10.1086/340095[11]Freud, P.G. (1970) Physics: A Contemporary Perspective.Taylor and Francis Group.[12]Khinchin, A.I. (1949) Mathamatical Foundation of statis-tical mechanics. Dover Publications, New York.[13]Frampton, P., Stephen, D.H., Kephar, T.W. and Reeb, D.(2009) Classical Quantum Gravity. 26, 145005.doi:10.1088/0264-9381/26/14/145005All Rights Reserved.。
关于Spherical Harmonic

学习笔记--关于Spherical Harmonic xheartblue 2006-2-4关键字:Spherical Harmonic 球面调和函数 球面谐波函数 球形调和函数关联勒让德方程 勒让德多项式 正交多项式 正交函数系Spherical Harmonic 在图形学里,准确的说应该是高级光照技术里, 最近恐怕比较流行. 出于赶时髦的原因,我也在看,无奈数学基础太差了. 只好几乎把所有的时间全用在数学上了.从基本的数学分析看到泛函分析. 名词听的多了,渐渐也对Spherical harmonic 是什么玩意有了一点点了解. 了解而已, 不是理解..写出来, 整理整理思路而已. 望还不了解Spherical Harmonic 何物而来看本文的人自己能带着鄙视的眼光去理解. 否则如果本人理解错误而带坏了小孩概不负责 特此申明.Spherical harmonic 翻译成中文应该叫 球面调和函数, 是调和函数的一种. 所谓调和函数是一类函数(好像是废话). 满足Laplace 方程的的函数u 称为harmonic function. harmonic function 据说有一些很特殊的性质(偶还不了解是什么性质) , 于是有了harmonic analysis 这样的的数学分支.02=∇u 在极坐标系中把拉普拉斯方程表示成极坐标的形式,然后进行分离变量. (这个过程实在是太乱了,打个公式要半天, 具体见工程数学: 数学物理方程和特殊函数). 我们在求解这个方程的时候,会得到一个只和θ有关的方程0sin )1(cot 2222=Θ⎥⎦⎤⎢⎣⎡+++Θ+Θθθθθm n n d d d d 这个方程称为连带勒让德方程或者关联勒让德方程 – Associated Legendre Equation (恩,看来是个法国进口的方程.洋货啊, 看起来都复杂一些) . 给这个方程加一些条件,可以得到一些解. 其中有一些就叫勒让德多项式. 勒让德多项式是正交的.所谓正交的. 表示这个玩意满足 .)01(,其他都为是为j i P P j i j i ==•δ从实变函数和泛函分析的课程可以知道, 在L2空间中, 函数可以展开成关于一组完备的正交函数集, 典型的傅立叶级数就是个例子. 并且展开形式为i i B c f ∑= 其中ci 为系数,在傅立叶级数中,这个就是所谓的傅立叶系数, Bi 为正交函数中的一个.其中.也就是函数在Bi 这个基(向量空间中的基)上的投影, 也就是函数空间中的内积运算. 这样我们可以把任何一个函数都展开成级数.当然这个函数是要满足一定条件的.比如平方可积. ∫×=Bi f c i现在我们回到球面上来. 构造正交函数系ϕθπϕθim m l m l e P m l m l l Y )(cos )!()!(412),(+−+≡. 这玩意就是前面那个Laplace 方程一个解,也就是传说中的spherical harmonic. 那个P 呢,就是勒让德多项式了. 可以验证.他是一个正交的函数系. 而且是完备的. 用它.我们可以把球面上任何一个函数展开成以ϕθπϕθim m l m l e P m l m l l Y )(cos )!()!(412),(+−+≡为基的级数.. 当然,在球面上的遮挡关系也是可以这样的函数 , 球面上各个方向的辐射强度也是这个这样的函数, 同样次表面散射的能力也是个这样的函数, 理论上我们可以把这些函数用spherical harmonic 精确的还原出来. 而我们只需要记录那个Ci就可以了… 不过Ci是无穷多个. 出于人道主义,在一般的real-time rendering中,似乎Ci的个数是16 . 这也就是spherical harmonic 光照,不能模拟高频场景的一个原因了. 同时Ci的计算是比较复杂的. 这样很容易理解为什么DX9里的那几个demo的预处理跟乌龟一样.其实Ci的计算除了慢以外, 还很有技巧, 具体见<Advanced.Lighting.and.Materials.With.Shaders>的相关章节, 准确说是第8章.而关于spherical harmonic则见….. 偶还没有这样的书.不过<数学物理方程和特殊函数>中,有关于勒让德多项式的详细章节了.同样作为工程数学, 它还教你怎么用这个东西来近似球面上的一些函数,,, 比如电荷分布之类的. 类推到图形学上 …. 呵呵, 不说了. 实在没怎么看明白.参考书籍和资料/SphericalHarmonic.html关于spherical harmonic的.<工程数学: 数学物理方程和特殊函数> 高等教育出版社南京工学院编著关于Legendre Polynomial的.<函数论和泛函分析初步> 关于L2空间和L2空间上的傅立叶变换和傅立叶级数<Advanced Lighting and Materials With Shaders > 专门介绍高级光照的(又是废话,看书名就知道) 有人说这本书不好. 不过我觉得似乎这本书里讲SH是讲的最清楚的. 本着厚道的原则,顶一下.。
steinharmonic analysis

steinharmonic analysisStein's harmonic analysis, developed by mathematician Elias M. Stein, is a powerful mathematical tool used to study functions in signal processing, image analysis, and various other areas of mathematics. In this article, we will explore the key concepts and techniques involved in Stein's harmonic analysis.Harmonic analysis is a branch of mathematics that deals with the representation and decomposition of functions as a sum of simpler periodic functions called harmonics. Stein's harmonic analysis focuses on the study of functions and their properties using harmonic analysis techniques.One of the fundamental concepts in Stein's harmonic analysis is the Fourier transform. The Fourier transform of a function is a mathematical operation that decomposes the function into a collection of sinusoidal functions with varying frequencies and amplitudes. This decomposition provides valuable information about different components of the function, which can be useful in understanding and analyzing signals.The Fourier transform can be visualized as a way to transform afunction from the time/space domain to the frequency domain. In the frequency domain, the function is represented as a sum of sinusoidal functions, each associated with a particular frequency. This transformation allows us to analyze the function in terms of its frequency content, enabling us to identify important features and patterns that may be hidden in the time/space domain representation.Stein's harmonic analysis builds upon the Fourier transform by introducing techniques such as wavelet analysis andtime-frequency analysis. Wavelet analysis is a mathematical tool used to analyze signals at different resolutions. Unlike the Fourier transform, which provides a global frequency analysis, wavelet analysis allows for a localized analysis of the frequency components of a signal. This can be particularly useful when dealing with signals that contain transient or localized events.Time-frequency analysis, on the other hand, focuses on analyzing how the frequency content of a signal changes over time. This can be achieved using techniques such as the short-time Fourier transform or the spectrogram. These methods provide a representation of the signal that captures both its time andfrequency properties, allowing for a detailed analysis of transient events and changes in frequency content.Stein's harmonic analysis also incorporates concepts from functional analysis, which deals with the study of vector spaces of functions and operators. Functional analysis provides a rigorous mathematical framework for studying the properties and behavior of functions and operators in a more general context.One of the key contributions of Stein's harmonic analysis is the development of theory and techniques for dealing with non-linear and time-varying systems. Traditional harmonic analysis techniques often assume linearity and time-invariance, which may not hold in many practical applications. Stein's approach allows for the analysis of more complex systems that exhibit non-linear and time-varying behavior.In conclusion, Stein's harmonic analysis is a valuable mathematical tool for studying functions and their properties in various fields, including signal processing and image analysis. The concepts and techniques involved, such as Fourier transforms, wavelet analysis,and time-frequency analysis, provide powerful tools for analyzing and understanding the frequency content and behavior of functions. By incorporating ideas from functional analysis, Stein's approach also allows for the analysis of more complex andnon-linear systems. Overall, Stein's harmonic analysis has proven to be a versatile and powerful tool in the field of mathematics.。
SPSS词汇大全(中英文对照)复习课程
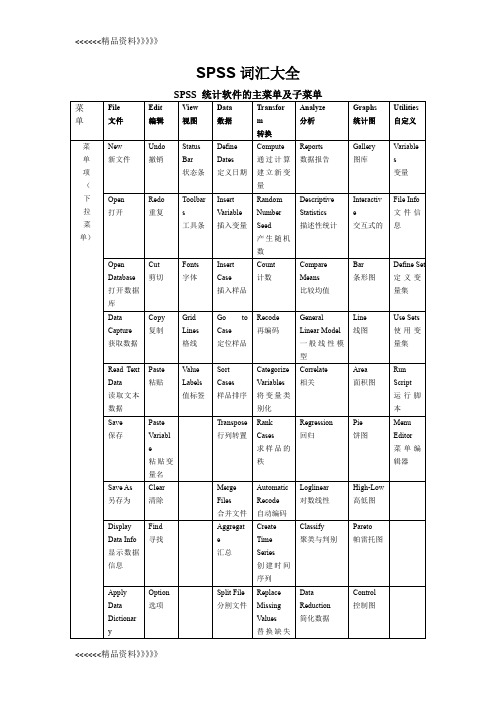
SPSS词汇(中英文对照)Absolute deviation, 绝对离差Absolute number, 绝对数Absolute residuals, 绝对残差Acceleration array, 加速度立体阵Acceleration in an arbitrary direction, 任意方向上的加速度Acceleration normal, 法向加速度Acceleration space dimension, 加速度空间的维数Acceleration tangential, 切向加速度Acceleration vector, 加速度向量Acceptable hypothesis, 可接受假设Accumulation, 累积Accuracy, 准确度Actual frequency, 实际频数Adaptive estimator, 自适应估计量Addition, 相加Addition theorem, 加法定理Additivity, 可加性Adjusted rate, 调整率Adjusted value, 校正值Admissible error, 容许误差Aggregation, 聚集性Alternative hypothesis, 备择假设Among groups, 组间Amounts, 总量Analysis of correlation, 相关分析Analysis of covariance, 协方差分析Analysis of regression, 回归分析Analysis of time series, 时间序列分析Analysis of variance, 方差分析Angular transformation, 角转换ANOVA (analysis of variance), 方差分析ANOVA Models, 方差分析模型Arcing, 弧/弧旋Arcsine transformation, 反正弦变换Area under the curve, 曲线面积AREG , 评估从一个时间点到下一个时间点回归相关时的误差ARIMA, 季节和非季节性单变量模型的极大似然估计Arithmetic grid paper, 算术格纸Arithmetic mean, 算术平均数Arrhenius relation, 艾恩尼斯关系Assessing fit, 拟合的评估Associative laws, 结合律Asymmetric distribution, 非对称分布Asymptotic bias, 渐近偏倚Asymptotic efficiency, 渐近效率Asymptotic variance, 渐近方差Attributable risk, 归因危险度Attribute data, 属性资料Attribution, 属性Autocorrelation, 自相关Autocorrelation of residuals, 残差的自相关Average, 平均数Average confidence interval length, 平均置信区间长度Average growth rate, 平均增长率Bar chart, 条形图Bar graph, 条形图Base period, 基期Bayes' theorem , Bayes定理Bell-shaped curve, 钟形曲线Bernoulli distribution, 伯努力分布Best-trim estimator, 最好切尾估计量Bias, 偏性Binary logistic regression, 二元逻辑斯蒂回归Binomial distribution, 二项分布Bisquare, 双平方Bivariate Correlate, 二变量相关Bivariate normal distribution, 双变量正态分布Bivariate normal population, 双变量正态总体Biweight interval, 双权区间Biweight M-estimator, 双权M估计量Block, 区组/配伍组BMDP(Biomedical computer programs), BMDP统计软件包Boxplots, 箱线图/箱尾图Breakdown bound, 崩溃界/崩溃点Canonical correlation, 典型相关Caption, 纵标目Case-control study, 病例对照研究Categorical variable, 分类变量Catenary, 悬链线Cauchy distribution, 柯西分布Cause-and-effect relationship, 因果关系Cell, 单元Censoring, 终检Center of symmetry, 对称中心Centering and scaling, 中心化和定标Central tendency, 集中趋势Central value, 中心值CHAID -χ2 Automatic Interaction Detector, 卡方自动交互检测Chance, 机遇Chance error, 随机误差Chance variable, 随机变量Characteristic equation, 特征方程Characteristic root, 特征根Characteristic vector, 特征向量Chebshev criterion of fit, 拟合的切比雪夫准则Chernoff faces, 切尔诺夫脸谱图Chi-square test, 卡方检验/χ2检验Choleskey decomposition, 乔洛斯基分解Circle chart, 圆图Class interval, 组距Class mid-value, 组中值Class upper limit, 组上限Classified variable, 分类变量Cluster analysis, 聚类分析Cluster sampling, 整群抽样Code, 代码Coded data, 编码数据Coding, 编码Coefficient of contingency, 列联系数Coefficient of determination, 决定系数Coefficient of multiple correlation, 多重相关系数Coefficient of partial correlation, 偏相关系数Coefficient of production-moment correlation, 积差相关系数Coefficient of rank correlation, 等级相关系数Coefficient of regression, 回归系数Coefficient of skewness, 偏度系数Coefficient of variation, 变异系数Cohort study, 队列研究Column, 列Column effect, 列效应Column factor, 列因素Combination pool, 合并Combinative table, 组合表Common factor, 共性因子Common regression coefficient, 公共回归系数Common value, 共同值Common variance, 公共方差Common variation, 公共变异Communality variance, 共性方差Comparability, 可比性Comparison of bathes, 批比较Comparison value, 比较值Compartment model, 分部模型Compassion, 伸缩Complement of an event, 补事件Complete association, 完全正相关Complete dissociation, 完全不相关Complete statistics, 完备统计量Completely randomized design, 完全随机化设计Composite event, 联合事件Composite events, 复合事件Concavity, 凹性Conditional expectation, 条件期望Conditional likelihood, 条件似然Conditional probability, 条件概率Conditionally linear, 依条件线性Confidence interval, 置信区间Confidence limit, 置信限Confidence lower limit, 置信下限Confidence upper limit, 置信上限Confirmatory Factor Analysis , 验证性因子分析Confirmatory research, 证实性实验研究Confounding factor, 混杂因素Conjoint, 联合分析Consistency, 相合性Consistency check, 一致性检验Consistent asymptotically normal estimate, 相合渐近正态估计Consistent estimate, 相合估计Constrained nonlinear regression, 受约束非线性回归Constraint, 约束Contaminated distribution, 污染分布Contaminated Gausssian, 污染高斯分布Contaminated normal distribution, 污染正态分布Contamination, 污染Contamination model, 污染模型Contingency table, 列联表Contour, 边界线Contribution rate, 贡献率Control, 对照Controlled experiments, 对照实验Conventional depth, 常规深度Convolution, 卷积Corrected factor, 校正因子Corrected mean, 校正均值Correction coefficient, 校正系数Correctness, 正确性Correlation coefficient, 相关系数Correlation index, 相关指数Correspondence, 对应Counting, 计数Counts, 计数/频数Covariance, 协方差Covariant, 共变Cox Regression, Cox回归Criteria for fitting, 拟合准则Criteria of least squares, 最小二乘准则Critical ratio, 临界比Critical region, 拒绝域Critical value, 临界值Cross-over design, 交叉设计Cross-section analysis, 横断面分析Cross-section survey, 横断面调查Crosstabs , 交叉表Cross-tabulation table, 复合表Cube root, 立方根Cumulative distribution function, 分布函数Cumulative probability, 累计概率Curvature, 曲率/弯曲Curvature, 曲率Curve fit , 曲线拟和Curve fitting, 曲线拟合Curvilinear regression, 曲线回归Curvilinear relation, 曲线关系Cut-and-try method, 尝试法Cycle, 周期Cyclist, 周期性D test, D检验Data acquisition, 资料收集Data bank, 数据库Data capacity, 数据容量Data deficiencies, 数据缺乏Data handling, 数据处理Data manipulation, 数据处理Data processing, 数据处理Data reduction, 数据缩减Data set, 数据集Data sources, 数据来源Data transformation, 数据变换Data validity, 数据有效性Data-in, 数据输入Data-out, 数据输出Dead time, 停滞期Degree of freedom, 自由度Degree of precision, 精密度Degree of reliability, 可靠性程度Degression, 递减Density function, 密度函数Density of data points, 数据点的密度Dependent variable, 应变量/依变量/因变量Dependent variable, 因变量Depth, 深度Derivative matrix, 导数矩阵Derivative-free methods, 无导数方法Design, 设计Determinacy, 确定性Determinant, 行列式Determinant, 决定因素Deviation, 离差Deviation from average, 离均差Diagnostic plot, 诊断图Dichotomous variable, 二分变量Differential equation, 微分方程Direct standardization, 直接标准化法Discrete variable, 离散型变量DISCRIMINANT, 判断Discriminant analysis, 判别分析Discriminant coefficient, 判别系数Discriminant function, 判别值Dispersion, 散布/分散度Disproportional, 不成比例的Disproportionate sub-class numbers, 不成比例次级组含量Distribution free, 分布无关性/免分布Distribution shape, 分布形状Distribution-free method, 任意分布法Distributive laws, 分配律Disturbance, 随机扰动项Dose response curve, 剂量反应曲线Double blind method, 双盲法Double blind trial, 双盲试验Double exponential distribution, 双指数分布Double logarithmic, 双对数Downward rank, 降秩Dual-space plot, 对偶空间图DUD, 无导数方法Duncan's new multiple range method, 新复极差法/Duncan新法Effect, 实验效应Eigenvalue, 特征值Eigenvector, 特征向量Ellipse, 椭圆Empirical distribution, 经验分布Empirical probability, 经验概率单位Enumeration data, 计数资料Equal sun-class number, 相等次级组含量Equally likely, 等可能Equivariance, 同变性Error, 误差/错误Error of estimate, 估计误差Error type I, 第一类错误Error type II, 第二类错误Estimand, 被估量Estimated error mean squares, 估计误差均方Estimated error sum of squares, 估计误差平方和Euclidean distance, 欧式距离Event, 事件Event, 事件Exceptional data point, 异常数据点Expectation plane, 期望平面Expectation surface, 期望曲面Expected values, 期望值Experiment, 实验Experimental sampling, 试验抽样Experimental unit, 试验单位Explanatory variable, 说明变量Exploratory data analysis, 探索性数据分析Explore Summarize, 探索-摘要Exponential curve, 指数曲线Exponential growth, 指数式增长EXSMOOTH, 指数平滑方法Extended fit, 扩充拟合Extra parameter, 附加参数Extrapolation, 外推法Extreme observation, 末端观测值Extremes, 极端值/极值F distribution, F分布F test, F检验Factor, 因素/因子Factor analysis, 因子分析Factor Analysis, 因子分析Factor score, 因子得分Factorial, 阶乘Factorial design, 析因试验设计False negative, 假阴性False negative error, 假阴性错误Family of distributions, 分布族Family of estimators, 估计量族Fanning, 扇面Fatality rate, 病死率Field investigation, 现场调查Field survey, 现场调查Finite population, 有限总体Finite-sample, 有限样本First derivative, 一阶导数First principal component, 第一主成分First quartile, 第一四分位数Fisher information, 费雪信息量Fitted value, 拟合值Fitting a curve, 曲线拟合Fixed base, 定基Fluctuation, 随机起伏Forecast, 预测Four fold table, 四格表Fourth, 四分点Fraction blow, 左侧比率Fractional error, 相对误差Frequency, 频率Frequency polygon, 频数多边图Frontier point, 界限点Function relationship, 泛函关系Gamma distribution, 伽玛分布Gauss increment, 高斯增量Gaussian distribution, 高斯分布/正态分布Gauss-Newton increment, 高斯-牛顿增量General census, 全面普查GENLOG (Generalized liner models), 广义线性模型Geometric mean, 几何平均数Gini's mean difference, 基尼均差GLM (General liner models), 一般线性模型Goodness of fit, 拟和优度/配合度Gradient of determinant, 行列式的梯度Graeco-Latin square, 希腊拉丁方Grand mean, 总均值Gross errors, 重大错误Gross-error sensitivity, 大错敏感度Group averages, 分组平均Grouped data, 分组资料Guessed mean, 假定平均数Half-life, 半衰期Hampel M-estimators, 汉佩尔M估计量Happenstance, 偶然事件Harmonic mean, 调和均数Hazard function, 风险均数Hazard rate, 风险率Heading, 标目Heavy-tailed distribution, 重尾分布Hessian array, 海森立体阵Heterogeneity, 不同质Heterogeneity of variance, 方差不齐Hierarchical classification, 组内分组Hierarchical clustering method, 系统聚类法High-leverage point, 高杠杆率点HILOGLINEAR, 多维列联表的层次对数线性模型Hinge, 折叶点Histogram, 直方图Historical cohort study, 历史性队列研究Holes, 空洞HOMALS, 多重响应分析Homogeneity of variance, 方差齐性Homogeneity test, 齐性检验Huber M-estimators, 休伯M估计量Hyperbola, 双曲线Hypothesis testing, 假设检验Hypothetical universe, 假设总体Impossible event, 不可能事件Independence, 独立性Independent variable, 自变量Index, 指标/指数Indirect standardization, 间接标准化法Individual, 个体Inference band, 推断带Infinite population, 无限总体Infinitely great, 无穷大Infinitely small, 无穷小Influence curve, 影响曲线Information capacity, 信息容量Initial condition, 初始条件Initial estimate, 初始估计值Initial level, 最初水平Interaction, 交互作用Interaction terms, 交互作用项Intercept, 截距Interpolation, 内插法Interquartile range, 四分位距Interval estimation, 区间估计Intervals of equal probability, 等概率区间Intrinsic curvature, 固有曲率Invariance, 不变性Inverse matrix, 逆矩阵Inverse probability, 逆概率Inverse sine transformation, 反正弦变换Iteration, 迭代Jacobian determinant, 雅可比行列式Joint distribution function, 分布函数Joint probability, 联合概率Joint probability distribution, 联合概率分布K means method, 逐步聚类法Kaplan-Meier, 评估事件的时间长度Kaplan-Merier chart, Kaplan-Merier图Kendall's rank correlation, Kendall等级相关Kinetic, 动力学Kolmogorov-Smirnove test, 柯尔莫哥洛夫-斯米尔诺夫检验Kruskal and Wallis test, Kruskal及Wallis检验/多样本的秩和检验/H检验Kurtosis, 峰度Lack of fit, 失拟Ladder of powers, 幂阶梯Lag, 滞后Large sample, 大样本Large sample test, 大样本检验Latin square, 拉丁方Latin square design, 拉丁方设计Leakage, 泄漏Least favorable configuration, 最不利构形Least favorable distribution, 最不利分布Least significant difference, 最小显著差法Least square method, 最小二乘法Least-absolute-residuals estimates, 最小绝对残差估计Least-absolute-residuals fit, 最小绝对残差拟合Least-absolute-residuals line, 最小绝对残差线Legend, 图例L-estimator, L估计量L-estimator of location, 位置L估计量L-estimator of scale, 尺度L估计量Level, 水平Life expectance, 预期期望寿命Life table, 寿命表Life table method, 生命表法Light-tailed distribution, 轻尾分布Likelihood function, 似然函数Likelihood ratio, 似然比line graph, 线图Linear correlation, 直线相关Linear equation, 线性方程Linear programming, 线性规划Linear regression, 直线回归Linear Regression, 线性回归Linear trend, 线性趋势Loading, 载荷Location and scale equivariance, 位置尺度同变性Location equivariance, 位置同变性Location invariance, 位置不变性Location scale family, 位置尺度族Log rank test, 时序检验Logarithmic curve, 对数曲线Logarithmic normal distribution, 对数正态分布Logarithmic scale, 对数尺度Logarithmic transformation, 对数变换Logic check, 逻辑检查Logistic distribution, 逻辑斯特分布Logit transformation, Logit转换LOGLINEAR, 多维列联表通用模型Lognormal distribution, 对数正态分布Lost function, 损失函数Low correlation, 低度相关Lower limit, 下限Lowest-attained variance, 最小可达方差LSD, 最小显著差法的简称Lurking variable, 潜在变量Main effect, 主效应Major heading, 主辞标目Marginal density function, 边缘密度函数Marginal probability, 边缘概率Marginal probability distribution, 边缘概率分布Matched data, 配对资料Matched distribution, 匹配过分布Matching of distribution, 分布的匹配Matching of transformation, 变换的匹配Mathematical expectation, 数学期望Mathematical model, 数学模型Maximum L-estimator, 极大极小L 估计量Maximum likelihood method, 最大似然法Mean, 均数Mean squares between groups, 组间均方Mean squares within group, 组内均方Means (Compare means), 均值-均值比较Median, 中位数Median effective dose, 半数效量Median lethal dose, 半数致死量Median polish, 中位数平滑Median test, 中位数检验Minimal sufficient statistic, 最小充分统计量Minimum distance estimation, 最小距离估计Minimum effective dose, 最小有效量Minimum lethal dose, 最小致死量Minimum variance estimator, 最小方差估计量MINITAB, 统计软件包Minor heading, 宾词标目Missing data, 缺失值Model specification, 模型的确定Modeling Statistics , 模型统计Models for outliers, 离群值模型Modifying the model, 模型的修正Modulus of continuity, 连续性模Morbidity, 发病率Most favorable configuration, 最有利构形Multidimensional Scaling (ASCAL), 多维尺度/多维标度Multinomial Logistic Regression , 多项逻辑斯蒂回归Multiple comparison, 多重比较Multiple correlation , 复相关Multiple covariance, 多元协方差Multiple linear regression, 多元线性回归Multiple response , 多重选项Multiple solutions, 多解Multiplication theorem, 乘法定理Multiresponse, 多元响应Multi-stage sampling, 多阶段抽样Multivariate T distribution, 多元T分布Mutual exclusive, 互不相容Mutual independence, 互相独立Natural boundary, 自然边界Natural dead, 自然死亡Natural zero, 自然零Negative correlation, 负相关Negative linear correlation, 负线性相关Negatively skewed, 负偏Newman-Keuls method, q检验NK method, q检验No statistical significance, 无统计意义Nominal variable, 名义变量Nonconstancy of variability, 变异的非定常性Nonlinear regression, 非线性相关Nonparametric statistics, 非参数统计Nonparametric test, 非参数检验Nonparametric tests, 非参数检验Normal deviate, 正态离差Normal distribution, 正态分布Normal equation, 正规方程组Normal ranges, 正常范围Normal value, 正常值Nuisance parameter, 多余参数/讨厌参数Null hypothesis, 无效假设Numerical variable, 数值变量Objective function, 目标函数Observation unit, 观察单位Observed value, 观察值One sided test, 单侧检验One-way analysis of variance, 单因素方差分析Oneway ANOVA , 单因素方差分析Open sequential trial, 开放型序贯设计Optrim, 优切尾Optrim efficiency, 优切尾效率Order statistics, 顺序统计量Ordered categories, 有序分类Ordinal logistic regression , 序数逻辑斯蒂回归Ordinal variable, 有序变量Orthogonal basis, 正交基Orthogonal design, 正交试验设计Orthogonality conditions, 正交条件ORTHOPLAN, 正交设计Outlier cutoffs, 离群值截断点Outliers, 极端值OVERALS , 多组变量的非线性正规相关Overshoot, 迭代过度Paired design, 配对设计Paired sample, 配对样本Pairwise slopes, 成对斜率Parabola, 抛物线Parallel tests, 平行试验Parameter, 参数Parametric statistics, 参数统计Parametric test, 参数检验Partial correlation, 偏相关Partial regression, 偏回归Partial sorting, 偏排序Partials residuals, 偏残差Pattern, 模式Pearson curves, 皮尔逊曲线Peeling, 退层Percent bar graph, 百分条形图Percentage, 百分比Percentile, 百分位数Percentile curves, 百分位曲线Periodicity, 周期性Permutation, 排列P-estimator, P估计量Pie graph, 饼图Pitman estimator, 皮特曼估计量Pivot, 枢轴量Planar, 平坦Planar assumption, 平面的假设PLANCARDS, 生成试验的计划卡Point estimation, 点估计Poisson distribution, 泊松分布Polishing, 平滑Polled standard deviation, 合并标准差Polled variance, 合并方差Polygon, 多边图Polynomial, 多项式Polynomial curve, 多项式曲线Population, 总体Population attributable risk, 人群归因危险度Positive correlation, 正相关Positively skewed, 正偏Posterior distribution, 后验分布Power of a test, 检验效能Precision, 精密度Predicted value, 预测值Preliminary analysis, 预备性分析Principal component analysis, 主成分分析Prior distribution, 先验分布Prior probability, 先验概率Probabilistic model, 概率模型probability, 概率Probability density, 概率密度Product moment, 乘积矩/协方差Profile trace, 截面迹图Proportion, 比/构成比Proportion allocation in stratified random sampling, 按比例分层随机抽样Proportionate, 成比例Proportionate sub-class numbers, 成比例次级组含量Prospective study, 前瞻性调查Proximities, 亲近性Pseudo F test, 近似F检验Pseudo model, 近似模型Pseudosigma, 伪标准差Purposive sampling, 有目的抽样QR decomposition, QR分解Quadratic approximation, 二次近似Qualitative classification, 属性分类Qualitative method, 定性方法Quantile-quantile plot, 分位数-分位数图/Q-Q图Quantitative analysis, 定量分析Quartile, 四分位数Quick Cluster, 快速聚类Radix sort, 基数排序Random allocation, 随机化分组Random blocks design, 随机区组设计Random event, 随机事件Randomization, 随机化Range, 极差/全距Rank correlation, 等级相关Rank sum test, 秩和检验Rank test, 秩检验Ranked data, 等级资料Rate, 比率Ratio, 比例Raw data, 原始资料Raw residual, 原始残差Rayleigh's test, 雷氏检验Rayleigh's Z, 雷氏Z值Reciprocal, 倒数Reciprocal transformation, 倒数变换Recording, 记录Redescending estimators, 回降估计量Reducing dimensions, 降维Re-expression, 重新表达Reference set, 标准组Region of acceptance, 接受域Regression coefficient, 回归系数Regression sum of square, 回归平方和Rejection point, 拒绝点Relative dispersion, 相对离散度Relative number, 相对数Reliability, 可靠性Reparametrization, 重新设置参数Replication, 重复Report Summaries, 报告摘要Residual sum of square, 剩余平方和Resistance, 耐抗性Resistant line, 耐抗线Resistant technique, 耐抗技术R-estimator of location, 位置R估计量R-estimator of scale, 尺度R估计量Retrospective study, 回顾性调查Ridge trace, 岭迹Ridit analysis, Ridit分析Rotation, 旋转Rounding, 舍入Row, 行Row effects, 行效应Row factor, 行因素RXC table, RXC表Sample, 样本Sample regression coefficient, 样本回归系数Sample size, 样本量Sample standard deviation, 样本标准差Sampling error, 抽样误差SAS(Statistical analysis system ), SAS统计软件包Scale, 尺度/量表Scatter diagram, 散点图Schematic plot, 示意图/简图Score test, 计分检验Screening, 筛检SEASON, 季节分析Second derivative, 二阶导数Second principal component, 第二主成分SEM (Structural equation modeling), 结构化方程模型Semi-logarithmic graph, 半对数图Semi-logarithmic paper, 半对数格纸Sensitivity curve, 敏感度曲线Sequential analysis, 贯序分析Sequential data set, 顺序数据集Sequential design, 贯序设计Sequential method, 贯序法Sequential test, 贯序检验法Serial tests, 系列试验Short-cut method, 简捷法Sigmoid curve, S形曲线Sign function, 正负号函数Sign test, 符号检验Signed rank, 符号秩Significance test, 显著性检验Significant figure, 有效数字Simple cluster sampling, 简单整群抽样Simple correlation, 简单相关Simple random sampling, 简单随机抽样Simple regression, 简单回归simple table, 简单表Sine estimator, 正弦估计量Single-valued estimate, 单值估计Singular matrix, 奇异矩阵Skewed distribution, 偏斜分布Skewness, 偏度Slash distribution, 斜线分布Slope, 斜率Smirnov test, 斯米尔诺夫检验Source of variation, 变异来源Spearman rank correlation, 斯皮尔曼等级相关Specific factor, 特殊因子Specific factor variance, 特殊因子方差Spectra , 频谱Spherical distribution, 球型正态分布Spread, 展布SPSS(Statistical package for the social science), SPSS统计软件包Spurious correlation, 假性相关Square root transformation, 平方根变换Stabilizing variance, 稳定方差Standard deviation, 标准差Standard error, 标准误Standard error of difference, 差别的标准误Standard error of estimate, 标准估计误差Standard error of rate, 率的标准误Standard normal distribution, 标准正态分布Standardization, 标准化Starting value, 起始值Statistic, 统计量Statistical control, 统计控制Statistical graph, 统计图Statistical inference, 统计推断Statistical table, 统计表Steepest descent, 最速下降法Stem and leaf display, 茎叶图Step factor, 步长因子Stepwise regression, 逐步回归Storage, 存Strata, 层(复数)Stratified sampling, 分层抽样Stratified sampling, 分层抽样Strength, 强度Stringency, 严密性Structural relationship, 结构关系Studentized residual, 学生化残差/t化残差Sub-class numbers, 次级组含量Subdividing, 分割Sufficient statistic, 充分统计量Sum of products, 积和Sum of squares, 离差平方和Sum of squares about regression, 回归平方和Sum of squares between groups, 组间平方和Sum of squares of partial regression, 偏回归平方和Sure event, 必然事件Survey, 调查Survival, 生存分析Survival rate, 生存率Suspended root gram, 悬吊根图Symmetry, 对称Systematic error, 系统误差Systematic sampling, 系统抽样Tags, 标签Tail area, 尾部面积Tail length, 尾长Tail weight, 尾重Tangent line, 切线Target distribution, 目标分布Taylor series, 泰勒级数Tendency of dispersion, 离散趋势Testing of hypotheses, 假设检验Theoretical frequency, 理论频数Time series, 时间序列Tolerance interval, 容忍区间Tolerance lower limit, 容忍下限Tolerance upper limit, 容忍上限Torsion, 扰率Total sum of square, 总平方和Total variation, 总变异Transformation, 转换Treatment, 处理Trend, 趋势Trend of percentage, 百分比趋势Trial, 试验Trial and error method, 试错法Tuning constant, 细调常数Two sided test, 双向检验Two-stage least squares, 二阶最小平方Two-stage sampling, 二阶段抽样Two-tailed test, 双侧检验Two-way analysis of variance, 双因素方差分析Two-way table, 双向表Type I error, 一类错误/α错误Type II error, 二类错误/β错误UMVU, 方差一致最小无偏估计简称Unbiased estimate, 无偏估计Unconstrained nonlinear regression , 无约束非线性回归Unequal subclass number, 不等次级组含量Ungrouped data, 不分组资料Uniform coordinate, 均匀坐标Uniform distribution, 均匀分布Uniformly minimum variance unbiased estimate, 方差一致最小无偏估计Unit, 单元Unordered categories, 无序分类Upper limit, 上限Upward rank, 升秩Vague concept, 模糊概念Validity, 有效性VARCOMP (Variance component estimation), 方差元素估计Variability, 变异性Variable, 变量Variance, 方差Variation, 变异Varimax orthogonal rotation, 方差最大正交旋转Volume of distribution, 容积W test, W检验Weibull distribution, 威布尔分布Weight, 权数Weighted Chi-square test, 加权卡方检验/Cochran检验Weighted linear regression method, 加权直线回归Weighted mean, 加权平均数Weighted mean square, 加权平均方差Weighted sum of square, 加权平方和Weighting coefficient, 权重系数Weighting method, 加权法W-estimation, W估计量W-estimation of location, 位置W估计量Width, 宽度Wilcoxon paired test, 威斯康星配对法/配对符号秩和检验Wild point, 野点/狂点Wild value, 野值/狂值Winsorized mean, 缩尾均值Withdraw, 失访Youden's index, 尤登指数Z test, Z检验Zero correlation, 零相关Z-transformation, Z变换。
自旋塞贝克系数的公式

自旋塞贝克系数的公式
自旋塞贝克系数(Sxy)是描述磁性材料中自旋热电效应的物理量,它定义为单位温差下磁化翻转产生的电动势的变化量与磁化翻转角度的变化量的比值。
其具体的数学表达式为:
Sxy = Δφ/ΔT
其中,Δφ代表磁化翻转导致的磁通量变化,ΔT代表温度的变化。
这个公式说明,自旋塞贝克系数Sxy实际上是磁化翻转角度变化对温度变化的导数。
换句话说,如果我们将磁化翻转视为一个过程,那么自旋塞贝克系数Sxy就是这个过程对温度变化率的导数。
需要注意的是,自旋塞贝克系数只在材料的磁化方向和外部磁场方向不一致时才有定义。
此外,自旋塞贝克效应是一种非常微弱的物理现象,其信号往往被材料的电阻、电容和电感等噪声所淹没。
因此,实际测量中需要采用特殊的技术手段来提取自旋塞贝克效应的信号。
希望这个解释能帮助你更好地理解自旋塞贝克系数的公式及其含义。
如果你还有其他问题或需要更深入的了解某个方面的信息,请随时告诉我。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
Darya Frolova, Denis Simakov, and Ronen Basri
2
Darya Frolova et al.
Low dimensional representations of lighting have been recently justified analytically in [1, 14]. These studies show that the set of all Lambertian reflectance functions (the mapping from surface normals to intensities) is, to an accurate approximation, low dimensional, and that this space is spanned by the low order spherical harmonics. Explicit spherical harmonic bases have been used to solve a number of important problems: object recognition [1], photometric stereo [2], reconstruction of moving shapes [16], and image rendering [15]. The introduction of spherical harmonic analysis provides a useful tool for handling complex illumination, but this pioneering work [1, 14] is incomplete in a practically important aspect: the analysis in [1, 14] is not easily generalized for the case of arbitrary object shapes and albedos. In this paper we consider the case of Lambertian reflectance allowing for attached, but not for cast shadows. Thus, our analysis is applicable to convex objects illuminated by arbitrary combinations of point and extended sources. We begin by showing that under distant lighting the average accuracy of spherical harmonic representations can be bound from below by a bound that is independent of the shape of the object. For this result we assume that lighting can be cast on an object from any direction with equal probability, and that the distribution of the intensity of lighting is independent of its direction. We further consider a second case in which lighting is illuminating the object only from above, and derive an expression that allows us to calculate the accuracy of the spherical harmonic representation in this case. While we consider a single expression for the harmonic basis there are studies that seek to build an optimal basis for every specific object or illumination. Ramamoorthi in [13] presents analytical construction of an optimal basis for the space of images. His analysis is based on spherical harmonics, and the images are taken under point light sources (uniformly distributed). The results of [13] are generalized and extended in [11, 12] for different illumination distributions and materials. While they consider specific object geometries, our goal is to bound from below the approximation accuracy for arbitrary objects. In the second part of our paper we analyze what happens if we relax the assumption of infinitely distant illumination, and show that spherical harmonics still provide a good basis even for fairly close light. We find what distance to the light can be considered infinite, as far as a spherical harmonic approximation is concerned. Our results show that although the approximation accuracy can be very bad for extremely close light, it rapidly increases as the distance to the light Байду номын сангаасrows and even at rather small distances we achieve quite a good accuracy. The assumption of infinitely distant light greatly simplifies the analysis of illumination effects, and so it is widely utilized in Computer Vision studies. While there are studies that incorporate near light effects (as in [7]), we are unaware of previous theoretical analysis of this factor. The paper is divided as follows. In Section 2 we briefly review the use of spherical harmonics to represent lighting and reflectance. In Section 3 we derive lower bounds on the accuracy of spherical harmonic representations for objects of arbitrary shape and albedos under infinitely distant lighting. Finally, in Section 4
1
Introduction
Methods for solving various Computer Vision tasks such as object recognition and 3D shape reconstruction under realistic lighting often require a tractable model capable of predicting images under different illumination conditions. It has been shown in [3] that even for the simple case of Lambertian (matt) objects the set of all images of an object under varying lighting conditions occupies a volume of unbounded dimension. Nevertheless many researchers observed that in many practical cases this set lies close to a low-dimensional linear subspace [4, 6, 18]. Low-dimensional representations have been used for solving many Computer Vision problems (e.g., [8, 10, 17]).
Dept. of Computer Science and Applied Math, The Weizmann Institute of Science, Rehovot 76100, Israel, {darya.frolova, denis.simakov, ronen.basri}@weizmann.ac.il
