Physics Review Motion with Constant Velocity物理评论运动以恒定速度
角动量守恒车轮实验 原理

角动量守恒车轮实验原理The conservation of angular momentum is a fundamental principle in physics that states the total angular momentum of a closed system remains constant over time. This concept is crucial in understanding the behavior of rotating objects, such as a spinning wheel on an axle. The conservation of angular momentum can be demonstrated through the popular physics experiment involving a spinning bicycle wheel mounted on a vertical axle. This experiment is not only educational but also fascinating to observe in action.角动量守恒是物理学中的基本原理,它规定闭合系统的总角动量随时间保持恻常不变。
这个概念在理解旋转物体的行为中至关重要,比如装在竖直轴上的旋转自行车轮就能证明角动量守恒。
这个实验不仅具有教育意义,而且观察起来十分有趣。
In the experiment, a bicycle wheel with its axis mounted vertically is set into rotation using a string wrapped around its rim. When the wheel is spinning, it is then suspended by holding onto the axle. Interestingly, when the bicycle wheel is initially spinning and tilted sideways, it exhibits a peculiar behavior – it starts rotating about avertical axis perpendicular to the axle. This unexpected motion is a result of the conservation of angular momentum, where the torque caused by gravity causes the axis of rotation to change.实验中,把轴装在竖直位置的自行车轮用绕在轮缘上的绳子旋转起来。
匀速圆周运动推导加速度的公式

匀速圆周运动推导加速度的公式英文版Title: Derivation of Acceleration Formula for Uniform Circular MotionUniform circular motion, a fundamental concept in physics, refers to the movement of an object along a circular path with a constant speed. This motion is characterized by the object's constant angular velocity and the resulting acceleration, known as centripetal acceleration, which acts towards the center of the circle. In this article, we will derive the formula for centripetal acceleration in uniform circular motion.Let's consider an object moving in a circular path with a radius r and a constant speed v. The object completes one revolution in a time period T, resulting in an angular velocity ω (omega) given by ω = 2π/T. The linear speed v is related to the angular velocity and the radius by the formula v = ωr.Now, let's focus on the acceleration of the object. Since the speed is constant, the tangential acceleration is zero. However, there is a radial or centripetal acceleration acting towards the center of the circle. This acceleration is responsible for keeping the object in circular motion.To derive the formula for centripetal acceleration, we need to consider the change in velocity over time. In uniform circular motion, the velocity vector changes direction but not magnitude. Let's consider a small change in the velocity vector Δv after a time Δt. This change in velocity is perpendicular to the original velocity vector and指向圆心。
Physics 2 Straight -Line Motion

(2)
(1)
(3)
In graph of velocity vs. time • Gives velocity at any time. • Slope gives acceleration.
2-3 Acceleration
Average acceleration
aav v 2 v1 t 2 t1 v t
x x x
2
x
1
t1
t
t2
t
In graph of position vs. time, the magnitude of average velocity with the sign is the slope of the straight line that joins the initial and final points
Act
Graph of distance position vs. time
• Gives location at any time. • Displacement is change in position. • Slope gives velocity.
Position at t=3.0, x(3) =1.0 m
Classical Mechanics: – Mechanics: the science of motion and its cause Kinematics: a description of motion Dynamics: the study of the cause of motion – Classical: • Not too fast (v << c) • Not too small (d >> atom) Most everyday situations can be described in these terms. -Path of baseball -Orbit of planets -etc...
Principles of Physics--03Newton' Law and applications
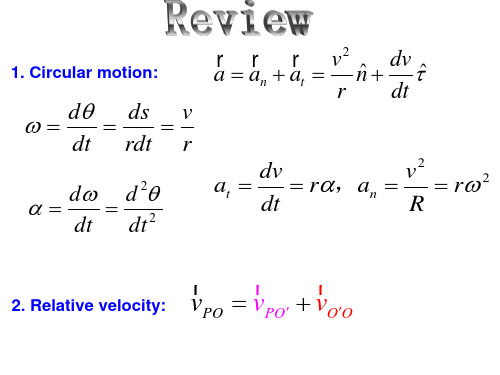
4. Solve the equations. 5. Check the result by introducing particular or extreme cases of quantities, and compare the results with your intuitive expectations.
§1 Newton’s First Law — Law of Inertia In the absence of external forces (or no net force), an object at rest remains at rest, and an object in motion continues in motion with a constant velocity (with a constant speed in a straight line). The acceleration of an body is zero when no force acts on it. An object has a tendency to maintain its original state of motion in the absence of a force. — This tendency is called inertia. Valid in the inertial frames. inertial frames —is one in which Newton’s first law is valid. Any reference frame that moves with a constant velocity wie is an inertial frame.
vPO = vPO′ + vO′O
发明相对论的英文名词解释

发明相对论的英文名词解释Einstein's Theory of Relativity: Unveiling the Secrets of the UniverseIntroductionThroughout history, groundbreaking scientific discoveries have revolutionized our understanding of the natural world. Few, however, can match the profound impact and enduring legacy of Albert Einstein's Theory of Relativity. This article aims to provide a comprehensive explanation and exploration of this groundbreaking theory, which forever changed the course of physics and our perception of space, time, and gravity.Understanding Relativity: A Paradigm Shift in PhysicsAlbert Einstein, a German-born theoretical physicist, introduced his Theory of Relativity in the early 20th century. It challenged and revolutionized the fundamental principles upon which classical physics was built. The theory consists of two pillars: the Special Theory of Relativity, formulated in 1905, and the General Theory of Relativity, established in 1915.Special Theory of Relativity: Spaceships, Time Dilation, and the Speed of LightThe Special Theory of Relativity is primarily concerned with objects moving at constant velocities in the absence of gravitational forces. Its foundation lies in two postulates: the principle of relativity and the constancy of the speed of light.The principle of relativity states that the laws of physics are the same for all observers in uniform motion. Consequently, motion becomes relative, and there is no absolute frame of reference. This principle contradicted the classical Newtonian mechanics that had dominated physics for centuries.Furthermore, the constancy of the speed of light is a fundamental tenet of Einstein's theory. According to this principle, the speed of light in a vacuum is always the same, regardless of the motion of the source or the observer. This assertion defied the common-sense notion that the speed of an object depends on the speed of the observer relative to the object.One of the most mind-boggling consequences of the Special Theory of Relativity is time dilation. As an object moves closer to the speed of light, time for that object slows down relative to a stationary observer. This phenomenon has been experimentally confirmed and is crucial for various practical applications, including space travel and particle accelerators.General Theory of Relativity: Gravity, Curved Spacetime, and the Fabric of the UniverseWhile the Special Theory of Relativity dealt with motion in the absence of gravity, the General Theory of Relativity expanded the framework to include gravity as a consequence of curved spacetime.According to Einstein's theory, mass and energy curve the fabric of spacetime, creating what we perceive as gravitational forces. This concept revolutionized our understanding of gravity, as opposed to the classical theory that described it simply as a force between objects.In the General Theory of Relativity, massive objects like stars and planets cause spacetime to curve, and other objects moving within this curved spacetime experience gravitational effects. This theory successfully explained various phenomena, such as the bending of light by massive objects and the precession of Mercury's orbit, which were previously inexplicable under classical physics.Implications and Applications of RelativityBeyond its profound impact on theoretical physics, the Theory of Relativity has had significant implications and practical applications in various fields.The development of GPS technology, for instance, heavily relies on both the Special and General Theories of Relativity. The precise coordination and synchronization of signals from satellites orbiting Earth require accurate timekeeping, which is affected bytime dilation due to the satellites' velocities and the gravitational fields they experience. Without taking these relativistic effects into account, GPS systems would rapidly become inaccurate.Furthermore, the confirmation of Einstein's theory during the 1919 solar eclipse, when Sir Arthur Eddington observed the apparent bending of starlight by the Sun's gravity, solidified the Theory of Relativity's status as a pillar of modern physics and gained worldwide attention. This monumental scientific discovery also captured the imagination of the public, propelling Einstein to international fame.ConclusionAlbert Einstein's Theory of Relativity stands as one of the most profound scientific achievements in human history. Its impact transcends the boundaries of physics, permeating various aspects of our lives. From our understanding of the fundamental workings of the cosmos to practical applications in technology, the Theory of Relativity has forever changed our perception of the universe. As we continue to probe the mysteries of the universe, the principles and implications of relativity remain at the forefront of scientific exploration.。
Physics 2 - Speed^J velocity and acceleration (in class) [自动保存的]
![Physics 2 - Speed^J velocity and acceleration (in class) [自动保存的]](https://img.taocdn.com/s3/m/b1b8af7ccf84b9d528ea7ad2.png)
So if that’s speed, what is
velocity?
Velocity :the speed of an object in a stated direction
ve
Or velocity is speed in a given direction.
Velocity is 25m/s due west
1. The total distance travelled between two points 2. the total time taken to travAettltbetween these two points
Distance measured in metres (m) Time measuredA in seconds (s)
– it has size and direction
Exercise page 26 2.13-2.15
Deceleration (retardation)
Deceleration is negative acceleration – the object is slowing down. Eg. – 4m/s2
for a body near to the Earth is constant
Supplement • Distinguish between speed and velocity • Define and calculate acceleration using time taken change of velocity • Calculate speed from the gradient of a distance-time graph • Calculate acceleration from the gradient of a speed-time graph • Recognise linear motion for which the acceleration is constant • Recognise motion for which the acceleration is not constant • Understand deceleration as a negative acceleration • Describe qualitatively the motion of bodies falling in a uniform gravitational field with and without air resistance (including reference to terminal velocity)
chap19(西尔斯当代大学物理)

Email: mdm@
mdm@
Welcome you send me mails to feedback anything about Teaching
index
• Part A Mechanism • Part B Electricity • Part C Magnetism
0 FB 0
B
maximum sideway force when
2
( FB ) max B qv
q
Fm 0
22
V
2. Hall effect:
B
Hall potential differenl l
BI n= VH el
可以证明上式不仅对矩形线圈成立对于均匀磁场中的任意形状的平面线圈也成立对于带电粒子在平面内沿闭合回路运动以及带电粒子自旋所具有的磁矩在磁场中受到的力矩都适用
Welcome you here for
University Physics
Meng Da Min HeFei University of Technology 2013 fall
B
20
Fast-Reading: example 19.12
21
Review:
1. Magnetic Fields:
FB qv B
FB Magnitude: B qv sin
direction: right-hand rule
F
B
q
v
zero-force axis (the direction of B )
Chap23 Electromagnetic waves
Chap 19 Magnetic Field and Magnetic Forces
物理专业词汇整理

专业词汇整理一.Subject name: (学科名称)1. Calculus微积分(学) mathematics 数学2.physics物理(学):statistical physics统计物理(学) modern physics 近代物理(学)Classic physics 经典物理学 molecular physics 分子物理学3.electromagnetism 电磁学 acoustics声学 kinematics 运动学4.mechanics力学 quantum mechanics 量子力学5. dynamics 动力学 thermodynamics 热力学6.general theory of relativity 广义相对论Special theory of relativity 狭义相对论二.在数学中的术语1.基本词汇:Function 函数(linear function 线性函数)equation 方程(linear equations 线性方程组)(注解:linear 是“线性”之意)formula 公式 theorem 定理(Pythagorean theorem 勾股定理)define 定义Projection 投影 test 检验 Determinant 行列式 minimum 最小值maximum 最大值2.矢量代数中的术语Vector 矢量(向量)①向量的几个概念:magnitude of vector 矢量的大小 projectionof vector 矢量的投影②向量的运算:subtraction of vector 矢量的减法 addition of vector 矢量的加法Dot (inner, scalar) product 点积(内积,标量积)Cross (vector) product 叉积(向量积)Scalar triple product(mixed product) 三重内积(混合积)③向量的分类:in the plane vector 平面向量 in space vector 空间向量(unit) Tangent vector (单位)切向量 (unit) normal vector (单位)法向量(注解:unit 有单位之意,而且与中文中的单位一样都有双重含义)Unit vector单位向量zero vector 零向量component vector(component of a vector )分向量Orthogonal vector 正交向量 rotating vector 旋转向量④**向量 position vector 位置矢量velocity vector 速度矢量displacement vector位移矢量 acceleration vector 加速度矢量3.级数中的术语series 级数①基本概念:sequence 序列(Fibonacci sequence 斐波那契数列)convergence 收敛性Term (数列中的)项②较为常见的级数:p _ series P—级数 harmonic series 调和级数power series幂级数alternating series交错级数Taylor series泰勒级数 Geometric series几何级数 position term series正项级数③收敛性与发散性convergence of series 级数的收敛性 partial sum 部分和Divergence of series 级数的发散性Absolute convergence (theorem) 绝对收敛(定理)conditional convergence 条件收敛radius of convergence 收敛半径interval of convergence 收敛区间iterated integral 逐次积分④检验法(审收法)nth_term test 第N项检验法limit comparison test极限比较检验Ratio test 比值检验Root test根植检验 alternating series test 交错级数审敛法geometric series test 几何级数审敛法 integral test 积分检验Basic comparison test 基本比较审敛法Rorschach test 罗氏检验⑤逼近法 Taylor’s approximation 泰勒逼近Maclaurin’s approximation 麦克劳林逼近4 微积分中的术语①基本概念:Variable变量 invariable 不变量domain of definition 定义域composite function复合函数parametric equation 参数方程 extreme 极值addition 加法subtraction 减法communicative law 交换律associative law 结合律②微分概念:differentiation 微分 chain rule 链式法则Derivative 导数:Directional derivative (angle) 方向导数(方向角)Partial derivative 偏导数 mixed second partial二阶混合偏导数second partial二阶偏导数 Lagrange multiplier 拉格朗日乘数③积分概念:integration积分 definitive integral定积分Riemann sum黎曼和improper integral 反常积分 integral test 积分检验inner limits 积分内限outer limits积分外限integration integral积分区间Initial condition 初始条件integration constant 积分常数integral curve 积分曲线 source function 原函数Upper bound of integral积分上限lower bound of integral积分下限multiple integral多重积分 double integral 二重积分triple integral 三重积分④ Newton-Leibniz formula 牛顿—莱布尼兹公式Fundament theorem of calculus微积分基本定理5 场论中的术语Field 场①基本概念:scalar field 标量场 operator 算子vector field(vector-valued function )矢量场(矢量函数)curl旋度 divergence散度 gradient(del)梯度 flux通量②积分:line integral线积分 surface integral面积分Independent of path 与路径无关③定理:Green’s theorem 格林定理Gauss’s theorem 高斯定理Stoker’s theorem 斯托克斯定理6.补充Limit极限 limits 范围continuous连续的 continuous everywhere处处连续coordinate system (coordinates)坐标系 quadrant 象限rectangular coordinates 直角坐标系cylinder coordinates 柱面坐标系spherical coordinates 球面坐标系Cartesian coordinates 笛卡尔坐标系Polar coordinates 极坐标系三物理中的常见术语1.force (力)and interaction(相互作用)Weak interaction弱相互作用 strong interaction强相互作用electromagnetic interaction 电磁相互作用Normal force法向力 tangential force切向力 drag force 阻力coriolis force科里奥利力 Lorenz force洛伦兹力elastic force弹性力 internal force 内力impulsive force 冲力inertial force惯性力 centrifugal inertial force 离心惯性力centre of force力心 force of gravity 重力friction force 阻力 static friction 静摩擦sliding friction 动摩擦2.constant (常量,常数)gas constant 气体普适常数 absolute constant 绝对常数damping constant 阻力常数gravitation constant引力常数3.inertial (惯性) inertial force惯性力 inertial mass 惯性质量noninertial frame 非惯性系inertial reference frame 惯性参考系rotational inertia 转动惯量w(定律) , equation(方程) ,formula(公式) and theorem(定理)kinematical equation 运动学方程Bernoulli’s equation 伯努利方程 poiseuill’s formula 泊肃叶公式theorem of momentum 动量定理theorem of kinetic energy 动能定理Theorem of angular momentum 角动量定理Parallel axis theorem 平行轴定理Perpendicular axis theorem 垂直轴定理conservation law of momentum 动量守恒定律conservation law of energy 能量守恒定律conservation law of angular momentum 角动量守恒定律conservation law of mechanical energy 机械能守恒定律Newton’s first/second/third law 牛顿三定律Law of universal gravitational force 万有引力定律Principle of superposition 叠加原理principle of work and energy 功能原理principle of relativity 相对性原理Hubble’s law 哈勃定律Hooker’s law 胡克定律Kepler’s law 开普勒定律konig’s theorem 克尼希定理Newton-Leibniz formula 牛顿—莱布尼兹公式Mass-energy equation(relation) 质能方程(关系)Temporal behavior 暂态行为 chaotic behavior 混沌行为Doppler effect 多普勒效应 Compton effect 康普顿效应Two-body problem 两体问题statically indeterminate problem 超静定问题5.unit(单位) and dimension(量纲)system of units单位制fundamental unit 基本单位derived unit 导出单位6.oscillation(振动) and wave(波)①波中的概念; wave front 波前wave speed 波速wave equation 波方程wave length 波长Wave number 波数wave shape波形wave resistance 波阻node波节 antinode 波腹②波的类型Forced oscillation受迫振动parametric oscillation参数动 infrasonic wave次声波 supersonic wave超声波 sound wave 声波electromagnetic wave电磁波 surface wave表面波 plane wave平面波 elastic wave弹性波 inelastic wave 非弹性波transverse wave横波 longitudinal wave 纵波mechanical wave机械波 simple harmonic wave(motion) 简谐波(简谐振动)solitary wave(sol ton)孤立波(弧子)pressure wave压力波 gravitational wave 引力波shock wave击波 cosine wave余弦波 sinusoidal wave正弦波standing wave驻波七 reference frame(参考系)Inertial reference frame 惯性参考系ceter-of-mass frame of reference质心参考系Moving reference frame 运动参考系Laboratory reference frame 实验室参考系basic reference frame 基本参考系八.Work(功) ,power(功率) and energy(能量)Element work 元功principle of work and energy 功能原理 mechanical energy 机械能conservation law of mechanical energy 机械能守恒定律conservation law of energy 能量守恒定律Potential energy 势能(位能) gravitational potential energy 引力势能Kinetic energy 动能 theorem of kinetic energy 动能定理Mass-energy relation(equation) 质能关系(质能方程)Mass-energy equivalence 质能等价性九.Velocity (速度),acceleration(加速度), coordinate(坐标)and motion(运动)Absolute velocity 绝对速度 relative velocity相对速度terminal velocity 终极速度First/second/third cosmic velocity 第一/二/三宇宙速度Instantaneous velocity/acceleration 瞬时速度/加速度Angular velocity/acceleration/coordinate 角速度/角加速度/角坐标Rectilinear motion with constant velocity 匀速直线运动Rectilinear motion with constant acceleration 匀加速直线运动Circular motion圆周运动 plane motion (translation)平面运动(平动)Rolling motion 滚动Linear accelerator直线加速器 cyclotron回旋加速器十.Momentum(动量) and angular momentum (角动量)Orbital angular momentum 轨道角动量theorem of momentum 动量定理Theorem of angular momentum 角动量定理conservation law of momentum 动量守恒定律conservation law of angular momentum 角动量守恒定律十一,常见的小词Temperature温度 position位置 displacement位移mass质量weight 重量experiment 实验liquid液体Pressure 压力particle 质点 simple pendulum 单摆atom原子 echo 回声error误差satellite人造卫星。
高一物理概念英语阅读理解20题

高一物理概念英语阅读理解20题1<背景文章>Newton's First Law, also known as the law of inertia, is a fundamental principle in physics. It states that an object at rest will remain at rest and an object in motion will continue in motion with a constant velocity in a straight line unless acted upon by an external force.Isaac Newton discovered this law through a series of careful observations and experiments. He realized that objects have a natural tendency to maintain their state of motion or rest.In real life, Newton's First Law has many applications. For example, when a car suddenly stops, the passengers in the car continue to move forward due to inertia. Another example is when a satellite is in orbit around the Earth. The satellite remains in motion because there is no significant external force acting on it.The significance of Newton's First Law lies in its fundamental nature. It helps us understand the behavior of objects in the absence of external forces and provides a basis for understanding more complex physical phenomena.1. Newton's First Law is also called ______.A. the law of gravityB. the law of inertiaC. the law of motionD. the law of energy答案:B。
A2_Physics_RevisionSummary

Circular Motion & Oscillationsy Angular speed, f t πθω2=ΔΔ=(assuming angle is a complete revolution, in radians) y Centripetal Force, rmv F 2=y Simple Harmonic Motion is a variation of displacement, velocity and acceleration withtime. It occurs when acceleration is proportional to displacement from a fixed point and in the opposition direction.o (assuming angle is a complete revolution, in radians) ()x f x a 222πω−=−=o θcos 0x x =(displacement at any point in the revolution) y Period of a pendulum, gl T π2= y Period of a mass on a string, km T π2= y These models assume a frictionless environment, which would allow perpetual motion. In reality damping will cause a loss of energy to the system which will over time reduce the maximum amplitudes. If the damping is too heavy there will be no oscillations at all. (Example: Shock Absorbers)y A force can be used to put this energy back into the system, this is known as forced oscillations. If the applied frequency of oscillation matches the natural frequency, then resonance will occur – where a large amount of energy is put into the system – which can be problematic for structural engineering, etc.Wavesy Waves may be transverse (displacement is perpendicular to direction of wave) orlongitudinal (displacement is in the same direction as the wave). The E-M spectrum is transverse waves, whilst sound and water waves are longitudinal.y Waves may be superimposed upon one another (addition or subtraction of amplitude usually if the frequency is the same).y Travelling/Progressive waves transmit energy from one place to another – no particles actually move apart from their displacement about a fixed point – but there is transfer of energy (the wave seemingly goes somewhere)y Standing/Stationary waves are the result of superposition of waves in such a way that the resultant wave does not transfer energy anywhere – there is no net displacement. o In a standing wave there are nodes (points of which there is no amplitude) and anti-nodes (points at which amplitude is at its peak).o Nodes and antinodes are always equidistant and located at half wavelength distances.y Wave speed, λf v =y For the E-M spectrum, wavespeed = speed of light, c .o Radio Waves (wavelength in metres) o Microwaves (wavelength in centimetres) o Infra Red (wavelength in micrometers)o Light (Visible) (wavelength from m) 77104107−−×⇒×o Ultraviolet (wavelength in nanometres) o X-Rays (wavelength around m) 101010−×o Gamma Rays (wavelength in picometers)o (Randy Max’s Intercourse Leaves Utterly Exhausted Girls)y Transverse waves may be polarised or unpolarised. Polarised waves move only in one plane about the axis along which the wave is directed. Unpolarised waves moves in all planes. Waves can be polarised with Polaroid filter or by using a fine grill which only certain planes of the wave can move through. Similarly polarised waves can be blocked with a grill.y Intensity, 24rPI π=(power per unit area, inverse square law)Superposition of Wavesy Graphical Superpositiony A wavefront is a line whereby all the points on it are in phase.y Single slit interference occurs when a wave travels through a slit and then spreads out. We can observe interference patterns, showing different parts of the wave are interacting with each other.y With double slit experiments, we see multiple sets of fringes in the interference pattern – due to superposition between the different beams of waves. The brightest points, maxima , are positioned equal distances apart.o Formula, Dxs=λ(x is maxima spacing, s is slit spacing, D is distance to screen)Quantum Phenomenay Energy of a Photon, (h is Plank constant)hf E =y The electron volt, the energy to move one coulomb across one volt.y Photoelectric effect… when photons hit a metal surface they provide an energy which can be used to release electrons from the material.o There are discrete energy states an electron may reside ato If the correct amount of energy is provided by a photon, an electron may move up an energy level (or more than one). If sufficient energy is provided to overcome the work function (the minimum energy required to release an electron from the surface of a material) a photoelectron may be emitted.o Threshold Frequency is the minimum wavelength required to cause the photoelectric effect.o The absorption/emission spectra of a material are related to the energy levels it has – since for each change in energy level a different energy photon must be absorbed.o Maximum energy of a photoelectron, ϕ−==hf E QV where q is charge on electron and V is stopping potential.o The photoelectric effect can be observed since the emission of electrons results in a change in charge (or if in a circuit, a current albeit a very small one).y Electrons can be diffracted (using a vacuum tube).y All objects have a wave-particle duality, which means we all have a wavelength. (de Broglie).o Wavelength, p h=λ(where p is momentum and h is the Plank constant)The Expanding Universey Stars have emission spectra which relates to their chemical composition. y A light year is the distance light will travel in one year.y The Doppler shift is where we observe spectral lines shifting depending whether an object is moving towards or away from us.o cv f f =Δ=Δλλ where v is the speed at which the object is moving o By measuring the observed frequency of light, and comparing this to the light given off by the same chemical the star is made of – in a lab environment – we can work out the shift in wavelength and thus use this to calculate the speed at which the galaxy is moving.o Note usually applied to galaxies not stars.o We can observe a red shift when we look at a galaxy, which indicates that most galaxies are moving away from, us. The balloon model shows us that this means the universe is expanding (but does not mean we are at its centre).y Hubble plots the distance a galaxy is from Earth against its velocity, the gradient of this curve is the hubble constant, H where Hd v =.o The inverse of the hubble constant is an approximate age of the universe. y There are three key possibilities for the future of the universe.o Open Universe Æ The universe continues expanding indefinitelyo Stable Universe Æ The universe will reach a maximum size then ceases to expand.o Closed Universe Æ The universe will eventually start collapsing in on itself. o These depend on the critical density of the universe, as to whether there is sufficient gravitational force to hold the universe together.Gravitational and Electric Fieldsy An electric field is a region where a charged particle experiences a force; similarly a gravitational field is a region where a particle with mass experiences a force.y Radial and Linear fields:+•Lines of equipotential are where the potential difference is the same in an electric field.In a uniform field the distance between lines of equipotential should be regular, so half way across the plate separation there will be half the voltage.•Comparing Electric and Gravitational Fields:E=• A Volt is defined as the energy required to move one Coulomb of charge. Thus QVCapacitance• Capacitance in Farads,CV Q =• Energy stored in a capacitor, 221CV E = • Equivalent capacitanceo In series, 3211111C C C C T ++=(since voltages add and charge is equal)o In parallel, 321C C C C T ++= (since charges add and voltage is equal) • There is exponential decay when discharging a capacitor though a resistor. • The area under a Current-Time graph is charge.Magnetic Fields & Electromagnetic Induction• Magnets have field lines too. A magnetic field is a region where a certain type of object experiences a force. • The flux density (field strength)(in Tesla) is defined, AB φ=where φ is magnetic flux• Force due to magnetic field, BIl F =o The direction is B is given by the left hand rule.o This can be re-arranged to BeV F =for a single electron. • Magnetic field can be measured with a Hall Probe. • In a solenoid (coil),nI B 0μ= ( n is number of coils)• About a single wire, rIB πμ20= ( r is perpendicular distance from wire)• An e.m.f. can be induced in a circuit by a changing the flux.• Induced e.m.f., tN ΔΔ−=φε• With a transformer, the flux is linked about a single coil, which makes a relationship between the emf on one side and emf on the other.o Sp S p N N V V =。
美国医学院入学测验(MCAT) Force And Motion 重点整理
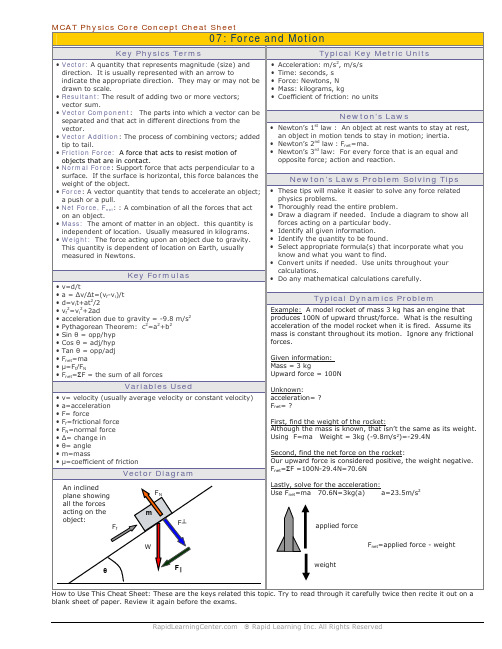
Vector Diagram
An inclined plane showing all the forces acting on the object: FN
m
Ff W F┴ applied force Fnet=applied force - weight
θ
F║
weight
How to Use This Cheat Sheet: These are the keys related this topic. Try to read through it carefully twice then recite it out on a blank sheet of paper. Review it again before the exams.
Key Formulas
• • • • • • • • • • • • • • • • • • • • • v=d/t a = Δv/Δt=(vf-vi)/t d=vit+at2/2 vf2=vi2+2ad acceleration due to gravity = -9.8 m/s2 Pythagorean Theorem: c2=a2+b2 Sin θ = opp/hyp Cos θ = adj/hyp Tan θ = opp/adj Fnet=ma μ=Ff/FN Fnet=ΣF = the sum of all forces
Newton’s Laws Pห้องสมุดไป่ตู้oblem Solving Tips
• These tips will make it easier to solve any force related physics problems. • Thoroughly read the entire problem. • Draw a diagram if needed. Include a diagram to show all forces acting on a particular body. • Identify all given information. • Identify the quantity to be found. • Select appropriate formula(s) that incorporate what you know and what you want to find. • Convert units if needed. Use units throughout your calculations. • Do any mathematical calculations carefully.
数学建模(西点军校资料)C1 (4)
Parachute Jumping, Falling, and Landing David C. Arney, Barbra S. Melendez , Debra Schnelle 1IntroductionIt is extremely important that leaders of airborne units understand the safety, medical, and operational issues involved in parachute jumping. Mathematical modeling of the flight and the landing of either a person or a cargo package can help with this understanding. In this section, we describe, analyze, and model several components of parachute jumping. These are basic models usingsimplified data in order to begin the study of these issues and should not be used for actual airborne operations. The Army has numerous technical manuals that govern the technical issues associated with airborne operations.The SituationAn airborne jumper, whose mass is 103 kg, leaps witha velocity of 0.555 m/s straight out (direction of the x -axis) from the side of an airplane which is moving at avelocity of 115 m/s (direction of the y -axis). Theairplane is flying at an altitude (height on the z -axis) of4000 meters. When the jumper leaves the aircraft, heis high enough in altitude that the air resistance can beconsidered negligible, and he falls as a freely fallingbody for 11.5 seconds until he pulls the parachutecord. After that point, the fall becomes a threedimensional projectile motion problem with non -constant acceleration along each axis as air resistancemust now be taken into account. The reference axesare established as follows (x -axis in direction of jumperleaving plane, y -axis in direction of plane when thejumper exits, z -axis is vertical, and the origin is on the ground directly under the plane the moment the jumperexits).When the parachute cord is pulled, we assume that the parachute deploys immediately and exerts a combined drag and air resistance force of magnitudeD = dv 2, where d is the drag coefficient due to the parachute and air resistance, and v is the velocity of the jumper. In this case, we use d = 20, but normally the value of d is difficult to determine, since it depends on so many variables. Assume the drag force is exerted only along the vertical direction (z-axis), and that the effect of air resistance along the y-direction can be modeled as a force of magnitude F = -bv , where the minus sign indicates that the force opposes the 1Formerly in Department of Physics, USMAdirection of motion and b is the drag coefficient due to air resistance. In this case, we use b = 10.Simultaneous to the opening of the parachute, the jumper experiences a cross wind in the positive x-direction of 1.2m/s. The force due to this cross windcan be modeled as G = b(w - v), whereb is the drag coefficient due to airresistance (b is set to 10 above), v is thespeed of the jumper, and w is the crosswind speed.The collision between the jumper andthe ground can also be modeled usingmathematics and physics. There areseveral medical and safety factors that need to be considered for such a collision. Rapid deceleration of the head can be very serious due to the sheer strain on the brain stem (shear means that the shape of an object has changed as the result of forces). A measure of this shear strain to the head is the severity index I , which is determined by the equationI = [2(v) / (g∆t)]2.5(∆t),where v is the velocity at impact, ∆t is the duration of impact, and g is the acceleration due to gravity. When the severity index for a collision is above 1000, the collision is fatal. When the value of the severity index is approximately 400, unconsciousness and mild concussion are the result.The stress on the long bones of the legs during the collision is compressive. When the compression force per unit area exceeds the ultimate tensile strength of the bone (given below for various bones, with their associated cross-sectional areas), the bone breaks in compression.Bone Ultimate Tensile AverageStrength Cross-Sectional(N/m2) Area (m2)Femur 1.21 x 108 5.81 x 10-4Tibia 1.40 x 108 3.23 x 10-42.20 x 108 4.42 x 10-4Spinal Cord(back)Spinal Cord1.80 x 108 4.42 x 10-4(neck)Table 1The compressive force F experienced as a result of the impact on the earth can be determined from a physics model using momentum. Momentum p is defined as p = mv and the Force model is F = ∆p/∆t, where ∆p is the change in momentum and ∆t is the time duration of the collision.If the jumper lands on the ground, we assume that he performs a perfect "parachute landing fall," as he was taught in his free fall class. The time of his impact will depend upon how soft the ground is where he lands. Snow or soft sand, for example, would significantly extend his duration of impact and thus reduce the force of impact.More Simplifying AssumptionsFor our discussion and the initial modeling of this situation, we use the following assumptions in addition to or amplifying those already discussed:•We are not considering the complexities of landing such as landing on uneven ground or in the trees or in an inappropriate manner.•We assume the parachute opens instantaneously and the drag force D = dv2takes effect immediately with no period of transition.•The jumper does not bend or twist upon landing, and thus the breaking torques of various bones and ligaments are not being considered.•The jumper leaves the airplane by jumping straight out with no rotation.•The information given for the severity index and the bone compression values for the tibia and femur are correct (although in reality they are approximate). •Even though the forces needed to provide the deceleration of the body produce great tensile stresses on ligaments and tendons and the failure level for these are much lower than for bone, we ignore these effects and concentrate on the bones.•The impact force is transmitted to the bones without attenuation through theligaments or muscles.Time and Path of the FallThe jumper waits 11.5 seconds before pulling hisparachute open. During this period of time, he isin free fall (no air resistance). His initial velocityin the vertical direction (z-axis) is zero, and theacceleration is due only to the gravitational forceof -9.80 m/s2. Thus, the jumper's vertical positionat the time he deploys his parachute is given byz (t) = z0+ v z0t+ 0.5 a z t 2 . Substitution producesz(11.5) = 4000 + 0.5 (-9.80) (11.5)2= 3351.975 m.His initial velocity in the x-direction is 0.555 m/s,and there is no acceleration in this direction (airresistance is negligible during the first 11.5seconds). So, his position in the x-direction is given by x(t) = x0+ v x0t+ 0.5 a x t 2. Substitution produces x(11.5) = 0 + 0.555(11.5) + 0= 6.3825 m.Similarly, the initial velocity in the y-direction is 115 m/s, and the acceleration in this direction is zero. Thus, the position equation in the y-direction is y = y0+ v y0t + 0.5 a y t2. Substitution produces y(11.5) = 0 + 115(11.5) + 0 = 1322.5 m. Therefore, the position coordinate at which the jumper opened his parachute is (x, y, z) = (6.3825, 1322.5, 3351.975) m.When the jumper pulls the parachute rip-cord, his velocity in the x- and y-directions will be the same as the initial values for those velocities when he leaped from the aircraft. His vertical velocity can be determined by the model v z= v z0+ a z t. Substitution produces v z= 0 + (-9.8)(11.5)= -112.7 m/s.Therefore, the velocity of the jumper when he pulls the parachute rip-cord is given by (v x, v y, v z) = (0.555, 115, -112.7) m/s.There are several ways to model the first few seconds of the parachute deployment. First, we have assumed that the chute deployment is instantaneous. In general, the jumper is falling very fast during the final phase of free fall and slows considerably to a constant (or terminal) velocity over some interval of time. For our situation, we will assume, based on an experiment, that this transition phase lasts 3 seconds and that during this transition, the jumper falls 53 meters downward.Now we determine the terminal (constant) velocity of the jumper when the parachute is deployed. He reaches terminal velocity when the drag force of the parachute, D = dv2, is equal to his weight, mg, so that the resultant acceleration is zero. In other words, when dv2 + mg = 0 the terminal (constant) velocity is reached. Solving for v, we get d= . Substitution produces,−v/mg=v= 7.1042 m/s in the downward direction.−(20/)8.9105−Since at terminal velocity the jumper is falling downward at a constant rate of7.1042 m/s, the time required to fall the remaining distance of 3298 meters is: (3298)/(7.1042) = 464.2 seconds. The total time of the fall is calculated by summing the time spent in free fall motion and the time with the parachute open, both before and after terminal velocity was reached. Then the total time equals 11.5 + 3.0 + 464.2 = 478.7 seconds.Determining the Landing CoordinatesApplying Newton's Second Law to the jumper's motion after the parachute deploys in both the x and y directions, we obtain the following models for the equations of motion.x-direction: F x = ma xwhere F = b(w - v)so, b(w-v) = ma xbw - bv = mama + bv = bwmx" + bx' = bw103x" + 10x' = 12.y-direction: F y= ma ywhere F = -bvso, - bv = ma yma + bv = 0my" + by' = 0103 y" + 10y' = 0 .Where m is the mass of the jumper, b is the coefficient of air resistance, and w is the wind speed. We solve these equations to obtain the position function. The initial conditions are the position and the velocity values at the time the parachute was opened.y-direction: Use the characteristic equation m r 2 + br = 0, with roots r = 0 and r = -(b/m). So, the form of the general solution is y(t) = C1e0 + C2e-(bt/m), where constants C1 and C2 are found by using the initial conditions.These conditions are at time t = 0; the time at which the parachute is opened and air resistance is no longer negligible. So at this time, y = 1322.5 m and v y= 115m/s (from above). Substitution produces: 1322.5 = C1 + C2and 115 = -(b/m) C2, where b = 10 kg/m and m = 103 kg. Therefore, C2 = - 1184.5 and C1 = 2507. The solution is written as y(t) = 2507 - 1184.5 e-(10/103)t.x-direction: In the x-direction, the differential equation is non-homogeneous, so the associated homogeneous equation mx" + bx' = 0 should be solved first, and then the non-homogeneous equation mx" + bx' = bw is solved. The characteristic equation for the homogeneous differential equation is mr 2+ br = 0 where the roots are: r = 0 and r = -(b/m). So, the complementary solution is: x c= C1e0+ C2 e-(bt/m). Now we solve for the particular solution, x p, of the non-homogeneous equation mx" + bx' = bw. We conjecture x p= At (the conjecture of x p = A does not work). Then, x p' = A, and x p" = 0. Substituting this solution into the differential equation yields m(0) + bA = bw. Therefore, A = w and x p= wt.The general solution is x = x c+ x p Therefore, for our non-homogeneous differential equation, the solution is x= C1 + C2 e-(bt/m)+ wt , and substitution of our parameter values produces x(t) = C1 + C2 e-(10/103)t+ 1.2 t.We use the initial condition to determine the constants C1and C2. Note that again these are the conditions at time t = 0, that is, the time at which the parachute opened and air resistance is no longer negligible. So, x(0) = 6.3825 m and v x(0)= 0.555 m/s (from above). This substitution produces 6.3825 = C1 + C2 and 0.555 = - (b/m) C2 + w. So, C2 = 6.6435 and C1 = - 0.261. We obtain the solutionx(t) = - 0.261 + 6.6435 e-(10/103)t+ 1.2 t.In order to find the position coordinates ofthe jumper's landing site, we use theposition equations and the total time of thejump. Given the position equations foundabove, simply substitute into theseequations the value of the time when thejumper lands (the total time experienced bythe man-parachute system), t = 467.2seconds. We find the landing position is:x = 560.4 m, y = 2507 m, and z = 0 m. The LandingWe need to determine the jumper's final velocity (i.e., his velocity immediately before impact) and the force of the collision upon the jumper. The velocity equations will be the derivatives, with respect to time, of the position equations. Therefore, we computey-direction: position equation ---> y(t) = 2507 - 1184.5 e-(10/103)tvelocity equation ---> v y(t) = (- 1184.5)(-10/103) e-(10/103)t= 115 e-(10/103)tx-direction:position equation ---> x(t) = - 0.261 + 6.6435 e-(10/103)t+ 1.2 tvelocity equation ---> v x(t) = (6.6435)(-10/103) e-(10/103)t+ 1.2= -0.645 e-(10/103)t+ 1.2At the time of impact, the jumper has reached terminal velocity in the z-direction. Therefore, we know v z(t) = - 7.1042 m/s. Since we determined the total time of the fall is 478.7 seconds, and he opened his parachute at 11.5 seconds, we are interested in his velocity at 467.2 seconds. This is the time value we substitute into the velocity equations above to obtain the velocity upon impact in each direction:v x(467.2) = -0.645 e-(10/103)(467.2) + 1.2 = 1.20 m/sv y(467.2) = 115 e-(10/103)(467.2) = 0.0 m/sv z(467.2) = - 7.1042 m/sIn vector notation, we write, v = v x i + v y j + v z k, so v = (1.20 i + 0 j - 7.1042 k )m/s.The magnitude of the velocity vector gives the speed of the jumper at the time of impact. In order to determine the speed we use the Pythagorean theorem: speed = (v x2 + v y2 + v z2)= (1.2)2 + (0)2+ (- 7.1042)2= 7.2048 m/s. When the jumper collides with the ground he experiences a change in momentum ∆p, given by p final -p initial. After the jumper collides with the ground, he has no momentum. Therefore, p final = 0. Additionally, if we assume that the collision takes place along a single direction, we can use the speed on impact to determine p initial. Momentum is given by p=mv, where m is the mass of the jumper and v is his velocity at impact. We can determine the initial momentum as p initial = mv = (103)(7.2048) = 742.0944 kg·m/s.Since the final momentum is 0, the change in momentum ∆p is 0 - 742.0944 =-742.0944 kg·m/s. This can also be described as 742.0944 N·s, where N = 1 kg·m/s2 indicates force in units of Newtons.We determine the force of impact using the equation F = ∆p /∆t . If we assume the jumper landed in thick bushes with a time of impact of 200 milliseconds (0.200 seconds), we calculate F = (742.0944) / (.200) = 3,710.472 N.Injuries?From the results we have calculated, we can determine if the jumper sustained any injuries. Table 1 gave us the ultimate tensile strength in N/m2 and the average cross-sectional area in m2 for four bones vulnerable to injury in this type of fall. By multiplying the tensile strength and the cross section we obtain the maximum force each bone is able to sustain without breaking. Table 2 provides this information.Bone Ultimate Tensile Average MaxStrength Cross-Sectional Sustainable(N/m2) Area (m2) Force (N) Femur 1.21 x 108 5.81 x 10-470301Tibia 1.40 x 108 3.23 x 10-445220Spinal Cord (back) 2.20 x 108 4.42 x 10-497240Spinal Cord (neck) 1.80 x 108 4.42 x 10-479560Table 2From Table 2 we see that a force of 3,710.47 N would not break any of the four bones analyzed.Finally, we check for the possibility of injury to the head by shear strain. Weuse the severity index, I, to determine possible injury. We calculateI = [2(v)/(g∆t)]2.5 (∆t) = [2( 7.2048) / [(9.8)(.200)] ]2.5 (.200)=29.310. The severity index of 29.310 is not close to the concussion level of 400. Therefore, we conclude that there should be no serious injuries to the head caused by this landing.ConclusionWe have used mathematical modeling in a parachute jump and landing scenario to analyze some of the factors associated with such an activity. Certainly, more detailed and sophisticated analyses could be performed to understand more of the components of parachute jumping. There are many issues that this scenario has ignored or that were assumed to hold that could use further study. For instance, the transition period from free fall to steady-state falling with a deployed chute contains numerous factors that are worthy of more study (see the exercises). Also, parachute deployment of heavy equipment like vehicles, weapon systems, and supplies is also worthy of study to insure the cargo lands safely and in the proper location.Exercises1. Are there additional assumptions being made that should be explicitly listed? What are they? Write a short essay that describes the role assumptions play in the modeling process.2. Use the severity Index formula to determine the shear strain on the head during the 3 second transition period from free fall to the chute-deployed steady-state constant velocity.3. What would happen if the jumper in the scenario landed on a very hard surface, like rocks, and the interval of impact was reduced to ∆t=0.01 seconds? Do you predict any injuries for the jumper?4. Solve for the landing time and location, if the same scenario and model are used with an exit altitude of 6,000 meters.5. Analyze the injury situation, if the same scenario and model are used with an exit altitude of 6,000 meters.6. In this scenario the transition phase from free-fall to steady-state descent with a fully deployed chute is 3 seconds with a altitude change of 53 meters. The velocity changes from 112.7 m/s to7.1 m/s over that interval. Will a linear model fit this data? Why or why not? Find a second-order (quadratic polynomial) to fit this data. AcknowledgementsThis scenario was developed from the following article:Melendez, Barbra and Schnelle, Debra, “Parachute Panic”, Interdisciplinary Lively Application projects (David Arney, editor), Washington: Mathematical Association of America, 1997.。
Physics Review Motion with Constant Velocity物理评论运动以恒定速度

What is the velocity of the pink car?
(A) -3 m/s (B) -1.5 m/s (C) 0 m/s (D) 1.5 m/s (E) 3 m/s
At what position do the cyclists meet up with each other?
What is the velocity of this object? (A) 0 m/s (B) 1 m/s (C) 2 m/s (D) 3 m/s (E) 4 m/s
A train starts out at the position 1,000 meters, and travels in the positive direction at 15 m/s.
What mathematical function correctly models the motion of the train as time goes on?
(A) x = (15 m/s) + (1,000 m)*t (B) x = (-15 m/s) + (1,000 m)*t (C) x = (1,000 m) + (15 m/s)*t (D) x = (1,000 m) – (15 m/s)*t (E) x = (-1,000 m) + (15 m/s)*t
When is the object at rest?
(A) Never (B) At t = 6 s (C) From t = 0 to t = 3 s (D) At t = 10 s (E) B and C are correct
When is the object moving the fastest?
物理中的合力 英语

物理中的合力英语The Concept of Resultant Force in Physics.In physics, the concept of resultant force, also known as the net force, is fundamental to understanding how objects move and interact with their environment. It represents the combined effect of all forces acting on an object, whether those forces are acting in the same direction or opposite directions. Understanding resultant force is crucial for comprehending Newton's laws of motion, which form the foundation of classical mechanics.Definition of Resultant Force.The resultant force is defined as the single force that would produce the same acceleration as the combination of all forces acting on an object. It is the vector sum of all forces, taking into account both magnitude and direction. In other words, it is the force that would replace all the individual forces acting on an object while producing thesame overall effect.Calculating Resultant Force.To calculate the resultant force, one must perform a vector addition of all the forces acting on an object. This involves breaking each force into its component parts (horizontal and vertical, for example) and then summing the components separately. The magnitude and direction of the resultant force can then be determined using the resulting component sums.If the forces are acting in the same direction, the resultant force is simply the sum of the magnitudes of the individual forces. However, if the forces are acting in opposite directions, the magnitudes are subtracted, and the direction of the larger force becomes the direction of the resultant force.Importance of Resultant Force.The concept of resultant force is crucial in physicsbecause it allows us to simplify complex systems bytreating multiple forces acting on an object as a single force. This simplification is essential for understanding and predicting the motion of objects.For example, when an object is at rest or moving with constant velocity, the resultant force acting on it is zero. This is because the forces acting on the object are balanced, meaning they cancel each other out. On the other hand, if the resultant force is not zero, it will cause the object to accelerate according to Newton's second law of motion (F=ma), where F is the resultant force, m is the mass of the object, and a is the acceleration.Applications of Resultant Force.The concept of resultant force finds applications in various fields of physics and engineering. Here are a few examples:1. Mechanics: In mechanics, the resultant force is used to determine the acceleration of objects under theinfluence of multiple forces. It is also essential for understanding how machines and structures behave under load.2. Projectile Motion: In projectile motion, theresultant force acting on a projectile (such as a ball thrown into the air) is gravity. By considering theresultant force, we can predict the path and range of the projectile.3. Statics: In statics, the study of objects at rest,the resultant force must be zero for an object to remain stationary. This principle is applied in the design of bridges and buildings to ensure they are stable and willnot collapse under the weight of loads.4. Dynamics: In dynamics, the study of objects in motion, the resultant force determines how an object's motion will change. It is crucial for understanding collisions, explosions, and other dynamic events.Conclusion.The concept of resultant force is fundamental to physics and provides a powerful tool for understanding and predicting the motion of objects. By considering the combined effect of all forces acting on an object, we can simplify complex systems and gain insights into how they behave. The applications of resultant force span various fields of physics and engineering, making it an essential concept in the scientific community.。
中考物理知识英语阅读理解20题

中考物理知识英语阅读理解20题1<背景文章>Newton's First Law, also known as the law of inertia, states that an object at rest will remain at rest and an object in motion will continue in motion with a constant velocity unless acted upon by an external force. This law was discovered by Sir Isaac Newton through his extensive studies and experiments.Newton observed that objects have a natural tendency to maintain their state of motion or rest. For example, a book lying on a table will stay there until someone picks it up or applies a force to it. Similarly, a moving car will continue to move in a straight line at a constant speed unless braked or steered by an external force.The applications of Newton's First Law are numerous in our daily lives. When we are sitting in a moving bus and the bus suddenly stops, we tend to lurch forward. This is because our bodies were in motion along with the bus and when the bus stops, there is an external force acting on it but our bodies tend to continue in motion due to inertia. Another example is when we throw a ball in space where there is no air resistance or other external forces acting on it. The ball will continue to move in a straight line at a constant speed until it collides with something.In conclusion, Newton's First Law is a fundamental principle of physics that helps us understand the behavior of objects in motion and at rest. It has wide applications in various fields such as engineering, astronomy, and sports.1. Newton's First Law is also called the law of ___.A. gravityB. inertiaC. magnetismD. electricity答案:B。
匀加速运动速度与位移的关系推导

匀加速运动速度与位移的关系推导英文版Derivation of the Relationship between Velocity and Displacement in Uniformly Accelerated MotionIn physics, uniformly accelerated motion refers to a type of motion in which an object travels with a constant acceleration. This acceleration remains the same throughout the duration of the motion. In this article, we will derive the relationship between velocity and displacement in uniformly accelerated motion.Let's start by defining some variables. Let's denote the initial velocity of the object as (v_0), the final velocity as (v), the acceleration as (a), and the displacement as (s). The time taken for the object to move from its initial position to its final position will be denoted as (t).The velocity-time equation for uniformly accelerated motion is given by:(v = v_0 + at)This equation tells us how the velocity of an object changes over time in uniformly accelerated motion.The displacement-time equation for uniformly accelerated motion is given by:(s = v_0t + \frac{1}{2}at^2)This equation relates the displacement of an object to the time it takes to travel that displacement in uniformly accelerated motion.To derive the relationship between velocity and displacement, we can rearrange the displacement-time equation. First, solve the velocity-time equation for (t): (t = \frac{v - v_0}{a})Now, substitute this expression for (t) into the displacement-time equation:(s = v_0 \left( \frac{v - v_0}{a} \right) + \frac{1}{2}a\left( \frac{v - v_0}{a} \right)^2)(s = \frac{v_0v - v_0^2}{a} + \frac{1}{2a}(v^2 - 2vv_0 +v_0^2))(s = \frac{v_0v - v_0^2}{a} + \frac{v^2 - 2vv_0 + v_0^2}{2a}) (s = \frac{2v_0v - 2v_0^2 + v^2 - 2vv_0 + v_0^2}{2a})(s = \frac{v^2 - v_0^2}{2a})This is the relationship between velocity and displacement in uniformly accelerated motion. It tells us that the displacement is equal to the difference between the final and initial velocities squared, divided by twice the acceleration.中文翻译匀加速运动速度与位移的关系推导在物理学中,匀加速运动指的是物体以恒定加速度进行的运动。
物理力学性质 英语作文

物理力学性质英语作文下载温馨提示:该文档是我店铺精心编制而成,希望大家下载以后,能够帮助大家解决实际的问题。
文档下载后可定制随意修改,请根据实际需要进行相应的调整和使用,谢谢!并且,本店铺为大家提供各种各样类型的实用资料,如教育随笔、日记赏析、句子摘抄、古诗大全、经典美文、话题作文、工作总结、词语解析、文案摘录、其他资料等等,如想了解不同资料格式和写法,敬请关注!Download tips: This document is carefully compiled by theeditor. I hope that after you download them,they can help yousolve practical problems. The document can be customized andmodified after downloading,please adjust and use it according toactual needs, thank you!In addition, our shop provides you with various types ofpractical materials,such as educational essays, diaryappreciation,sentence excerpts,ancient poems,classic articles,topic composition,work summary,word parsing,copyexcerpts,other materials and so on,want to know different data formats andwriting methods,please pay attention!Physics is a fascinating subject that explores the fundamental properties and behaviors of matter and energy. It encompasses a wide range of topics, from the study of motion and forces to the behavior of particles at the atomic and subatomic level. In this essay, I will discuss some of the interesting aspects of physics and its impact on our daily lives.One of the most basic concepts in physics is motion. Everything around us is in constant motion, whether it's the movement of cars on the road or the rotation of the Earth. Physics helps us understand the principles behind motion, such as Newton's laws of motion, which describe how objects move in response to forces. These laws can be applied to various situations, from calculating the trajectory of a projectile to understanding the behavior of objects in space.Another important aspect of physics is energy. Energyis the ability to do work, and it exists in many different forms, such as kinetic energy, potential energy, and thermal energy. Physics allows us to understand how energy is transferred and transformed, as well as how it can be conserved. For example, the study of thermodynamics helps us understand how heat flows and how it can be converted into other forms of energy, such as mechanical orelectrical energy.Physics also plays a crucial role in the development of technology. Many of the technological advancements we enjoy today, such as computers, smartphones, and renewable energy sources, are the result of breakthroughs in physics. For instance, the invention of transistors, which are fundamental components of electronic devices, was made possible by the understanding of quantum mechanics. Physics continues to push the boundaries of technology, leading to new discoveries and innovations that improve our lives.In addition to its practical applications, physics also has a profound impact on our understanding of the universe. Through the study of astrophysics and cosmology, scientistshave gained insights into the origins and evolution of the universe, as well as the nature of dark matter and dark energy. These discoveries challenge our existing knowledge and open up new avenues for exploration and discovery.In conclusion, physics is a fascinating field that encompasses a wide range of topics and has numerous applications in our daily lives. It helps us understand the fundamental properties and behaviors of matter and energy, and it plays a crucial role in the development of technology. Moreover, physics deepens our understanding of the universe and our place within it. So let's embrace the wonders of physics and continue to explore the mysteries of the world around us.。
初中物理 Kinematic Equations NIS grade11physics review
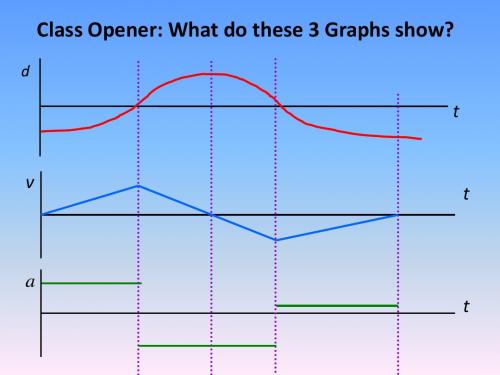
v
“forward area”
Area
t
“backward area”
The areas above and below are about equal, so even though a significant distance may have been covered, the displacement is about zero, meaning the stopping point was near the starting point. The position graph shows this too. d
• Motion is described by the equation d = vt • The slope (gradient) of the DT graph = Velocity • The steeper the line of a DT graph, the greater the velocity of the body
Calculus formulas
• Acceleration is the second derivative of displacement and velocity is the first derivative of displacement
• Integration will give the area under a curve
Grห้องสมุดไป่ตู้phing Tips
The same rules apply in making an acceleration graph from a velocity graph. Just graph the slopes! Note: a positive constant slope in blue means a positive constant green segment. The steeper the blue slope, the farther the green segment is from the time axis.
英语试题及其答案

英语试题及其答案第一套模拟题Part II Vocabulary and Structure(20%) (20minutes)Directions:There are 20 incomplete sentences in this part .For each sentence there are four choices marked A), B) ,C) ,and D).You must choose the oneanswer that best completes the sentence .Then mark the correspondingletter on the Answer Sheet with a single line through the center.13.Only in a few countries _D____a reasonable standard of living.A)the whole population enjoy C)do the whole population enjoyB)the whole population enjoys D)does the whole population enjoy14.We have ___A___to the government for a home improvement loanA)applied B)approached C)apologized D)appointed15.____C_,a man who expresses himself effectively is sure to succeed more rapidly than a man whose command of English is poor.(在其他相同的情况下)A)Were other things equal C)Other things being equalB)To be equal to other things D)Other things to be equal16.”May I speak to your manager Mr. Smith at four o’clock this afternoon?”“I’m sorry .Mr. Smith __B____to a conference before then.”A)has gone B)will have gone C)had gone D)would have gone17.There was a large crowd in the square _A____ against theIraq war.(反对)A)protesting B)protecting C)had gone D)all of these18.The writer has published many books, B_____are well received by the readers.A)all of whom B all of which C)all of them D)all of these19.Can you tell Mary _____B___ her twin sister?A) between B) from C) to D) with20.When the car crashed, your brother escaped being hurt, ______ ?A) didn’t it B) did it C) didn’t he D) did he21.The weather in China is different from_C___.A) that in America B) one in AmericaC) in America D) America22.“Would you mind opening the window?”“_B_____.”A) Y es, you may open B) Y es, pleaseC) No, of course not D) No, you can’t open now23.Judging from his manners at the party, he doesn’t seem _A____ much education. (一种猜测)A) to have received B) to receiveC) to have been received D) to be receiving24.I suggest _??__ on an excursion this Sunday.A) to go B) our going C) we are going D) us going25.Criticism and self-criticism is necessary __C___it helps us to correct our mistakes.A)until B)unless C)in that D)in which26.We need someone really___B__ who can organize the office and make it run smoothly.A)effective (有效地)B)efficient(效率高地) C)essentialD)executive27.Some of the experiments __D___ in our textbook aredifficult to performA)to describe B)be described C)describing D)described28.Tom graduated from a famous university at a very young age. He__A___have beenan outstanding student.A)must B)could C)should D)might29.Let’s hang up some nice paintings on these B_____walls of the great hall.(赤裸的)A)blank B)bare C)empty D)vacant30.The student in glasses confessed to _____the final English exam for another studentA)take B)taking C)have taken D)having taken31.We are going to have our office __A___ to make room fora new engineer.A) rearranged B) rearrange C) rearranging D) to rearrange32.Physics ___C__ with matter and motion.A) deal B) is C) deals D) arePart III Reading Comprehension (40%) (35minutes)Directions:There are four passages in this part. Each passage is followed by five questions or unfinished statements. For each question, there are four choices marked A), B), C) and D). You should choose the best answer. Then mark the corresponding letter on the Answer Sheet with a single line through the center.Passage OneQuestions 33 to 37 are based on the following passage.An earthquake is caused by a sudden slip on a fault(断层).A fault is a fracture in the crust(地壳)of the earth along which rock on one side have moved relative to those on the other side. Stresses in the earth’s outer layer push the sides of the fault together, build up and the rocks slips suddenly, releasing energyin waves that travel through the rock to cause the shaking that we feel during an earthquake.Earthquakes tend to be concentrated in narrow zones. There are 7 major crustal plates on earth, about 80 km thick, all in constant motion relative to one another. They move at between 10 and 130mm per year.It is estimated that there are several million earthquakes in the world each year. Many of these earthquakes go undetected because they occur in remote areas or have very small magnitudes(震级). The USGS Earthquake Information center locates 12,000 to 14,000 earthquakes each year (about 35 per day). On average, about 60 earthquakes per year are classified as significant, with 19 classified as major. A significant earthquake is one of magnitude 6.5 or higher or one of lesser magnitude that causes casualties (伤亡)or considerable damage. Major earthquakes have amagnitude larger than 7.0.33.The main idea of the passage is _____AA)how earthquakes are formed and classifiedB)how earthquakes can be predicted and locatedC)earthquakes do not so often occur on the earthD)earthquakes are considered to be a threat to humans34.Which of the following is NOT mentioned in this passage?DA)Earthquakes of large magnitudes cause casualties.B)Earthquakes often happen in narrow zones.C)Earthquakes involve the release of energyD)Earthquakes can cause waves in the sea.35.What can we learn form the passage?BA)Scientists can locate most earthquakesB)Earthquakes are very common on the earth.C)Scientists can find a way to prevent earthquakes.D)More earthquakes of higher magnitudes are detected than those of lower ones.36.How do scientists distinguish a significant earthquake from a major earthquake?CA)A significant earthquake is of higher magnitude.B)A significant earthquake can kill more people.C)A major earthquake is of higher magnitude.D)A major earthquake is of lower magnitude.37.The word ”fracture”(Line 1, paragraph 1)most probably means__D__.A)a stress in the crust C)an outer layer of the crustB)a large hole in the rock D)a cracked part in the rockPassage TwoQuestions 33 to 42 are based on the following passage.Have you ever suddenly felt that someone you know was in trouble —and he was? Have you ever dreamed something that came true later?Maybe you have a sixth sense (SS).This sense seems to let people know about events before they happen, or events that are happening some distance away.Here’s an example: A woman was ironing clothes. Suddenly she screamed, “My father is dead! I saw him sitting in the chair!” Just then, a telegram came. The woman’s father died of a heart attack. He died sitting in a chair.There are thousands of stories like this one on record. Scientists are studying them to find out what’s be hind these strange mental messages. Here’s another example —one of hundreds of dreams that have come true:A man dreamed he was walking along a road when a horseand carriage came by. The driver said,”There’s room for one. “The man felt the driver seemed d ead. So he ran away. The next day,the man was getting on a crowded bus. The bus driver said,”There’s r oom for one more. “ Then the man saw that the driver’s face was the same face he had seen in the dream. He wouldn’t get on the bus. As the bus droveoff, it crashed and burst into flames. Everyone was killed!Some people say stories like these are lies or coincidences —things that happenby chance. Others, including some scientists, say that the SS is real. From studies of it,we may someday learn more about the human mind.38.This article is mainly about__C__.A)the five senses B)the human dream C)the sixth sense D)the humanmind39.SS lets people know__A__.A)about events before they happen or happen some distance away.B)about events after they happen.C)about events that have come true.D)about events that are not happening or will happen some time later.40.By studying SS, scientists may get to_C____.A)learn how people tell lies.B)know more about human mind.C)learn how strange things happen.D)know more about human dreams.41.According to the passage, the author believes that the sixth sense is_C___.A)lifelike B)dreamlike C)in distance D)in existence42.In the last paragraph, the word “coincidences”probably means_A___.A)Things that happen by chanceB)Things that may not happenC)Things that must happenD)Things that happen in a dreamPassage ThreeQuestions 43 to 47 are based on the following passage.Information has always been at the center of human communication. Y ou mayask why. Well, communication between people involves giving and receiving information .The way we give and receive information today has experienced a revolution in the development of the mass media in the 20th century.The first truly mass communication medium was the newspaper. For the first time in history, people could read about events in their country and form around the world every day. However, there were two problems with newspapers to the countryside was a difficult and time-consuming task. Secondly, newspaper weren't always reliable, as there a limited range of opinions.Nowadays, we can choose from a wide variety of sources to get information. Television and the Internet have given us the chance to be informed about everything the minute it happens. Numerous radio and TV stations, satellite channels and millions of websites help people keep up with the latest news. People live history and are part of it.The media have come a long way in the last century and there is no doubt that we now live in the information age.Whatever type of me choose, it all comes down to the need for information. This will always be a basic need as long as communication is part of human nature /doc/c84639465.html,rmation is considered to be the center of human communication because_A.A) human communication means information exchangeB) human communication involves people's participationC) information is now experiencing a revolutionD) information helps people gather together44.What was the historical contribution of the newspaper asa sour of information?CA)It made the mass communication truly develop.B)It helped the mass communication develop in cities.C)It kept people timely informed about the world events.D)It kept reliable information available in big cities.45.What was NOT the problem with the newspaper of that timeDA)Its difficult delivery in the countryside.B)Its limitation of information sources.C)Its limited reliability of information.D)Its easy circulation in big cities.46.The third paragraph mainly tells us technology helpsA____.A) information easily availableB) people be part of historyC) Inform everything timelyD) Produce the latest news47.It can be safely concluded from the last paragraph that a basic need today is_B___.A)communication B)information C)high-technology D)media types Passage FourQuestions 48 to 52 are based on the following passage.Lately, plants that are usually only grown outdoors have found their way into inside spaces. There are a few simple reasons why this happens.The modern inside environment can be a good place for a wide variety of outdoor plants. Plants that ordinarily cannot grow in colder outdoor weather can be protected by the warmer inside spaces. Plants that used to be found only outside, such as trees, have become more and more popular as decorations (装饰)inside houses. Orchids (兰花), which were once only found in hot places, have begun to grow well in inside places.One reason that the modern home has made foreign outdoor plants more practical is the modern use of windows. In the past windows were used less often in buildings than they are today. It is partly because of the ability of large windows to admit a large quantity of light from the sun and to keep the heat in at the same time, that many outdoor plants have been able to make their move inside.It is true that most of the plants that have been successful in moving inside have been small. The best varieties are the small ones that make excellent decorations where other plants prove difficult to grow. Although some trees can be grown inside, it is not likely the larger varieties can be used. Unfortunately, most homes just don’t have the space to grow a monkey tree.48.The passage is mainly concerned with D________.A)the ability of large windowsB)the difficulty of moving monkey trees into homesC)the best suited treesD)growing plants inside houses49.Which of the following is most likely to be true? BA)The inside environment can not protect plants at all.B)People like to use plants as decorations in their houses.C)The uses of windows in the past were good for plants.D)People move plants into their houses to save them.50.Which of the following helps plants move indoors?DA)The modern use of windows.B)The modern use of inside space.C)The small varieties of plants inside.D)The foreign outdoor plants from hot places.51.According to the passage, modern windows are used toD_______.A)allow fresh air in B)grow plants and trees insideC)control the temperature D)provide heat and light52.It is not likely that large plants will move inside because C________.A)the weather is not suitableB)they cannot be movedC)there is not enough spaceD)they cannot be controlledPart ⅣTranslation from English into Chinese (10%) (15minutes) Direction:in this part, there are four items which you should translate into Chinese ,each item consisting of one sentence .Some of these sentences are taken from the reading passages you have just read in Part Ⅲof the Test Paper .You are allowed 15 minutes to do the translation. You can refer back to the passages so as to identify their meaning in the context. Write your translation on the Translation Sheet in Test Paper Two.T1.(Lines 1-2,Paragraph 2,Psassage1)There are 7 major crustal plates on earth, about 80 km thick, all in constant motion relative to one another.A)地球上有7种地壳结构,范围约80公里,它们的运动彼此相似。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
At t = B, the object is:
(A) not moving. (B) traveling at a constant speed. (C) speeding up. (D) slowing down. (E) turning around.
What is the velocity of the pink car?
(A) -3 m/s (B) -1.5 m/s (C) 0 m/s (D) 1.5 m/s (E) 3 m/s
At what position do the cyclists meet up with each other?
(A) 0 m (B) 5 m (C) 6 m (D) 8 m (E) They never meet
When do the cyclists have the same velocity?
(A) 0 s (B) 5 s (C) 6 s (D) 8 s (E) They never have the same velocity
What mathematical function correctly models the motion of the train as time goes on?
(A) x = (15 m/s) + (1,000 m)*t (B) x = (-15 m/s) + (1,000 m)*t (C) x = (1,000 m) + (15 m/s)*t (D) x = (1,000 m) – (15 m/s)*t (E) x = (-1,000 m) + (15 m/s)*t
At t = D, the object is:
(A) not moving. (B) moving in the positive direction. (C) moving in the negative direction. (D) speeding up. (E) slowing down.
Physics Review: Motion with Constant Velocity
How many times are the cars right next to one another?
(A) Zero (B) One (C) Two (D) Three (E) Not enough information to deterut at the position 1,000 meters, and travels in the negative direction at 15 m/s.
What will be the train’s position at t = 60 s?
(A) 1,900 m (B) 1,100 m (C) 900 m (D) 100 m (E) None of the above
What is the velocity of this object? (A) 0 m/s (B) 1 m/s (C) 2 m/s (D) 3 m/s (E) 4 m/s
A train starts out at the position 1,000 meters, and travels in the positive direction at 15 m/s.
When is the object at rest?
(A) Never (B) At t = 6 s (C) From t = 0 to t = 3 s (D) At t = 10 s (E) B and C are correct
When is the object moving the fastest?
