量子化学习题及答案
量子化学考试试题

量子化学考试试题一、选择题(每题 5 分,共 30 分)1、量子化学中,描述微观粒子运动状态的函数被称为()A 波函数B 概率密度C 哈密顿量D 薛定谔方程2、下列哪个量子数决定了原子轨道的形状()A 主量子数B 角量子数C 磁量子数D 自旋量子数3、对于氢原子的 1s 轨道,其电子出现概率最大的位置是()A 原子核处B 离核无穷远处C 离核一定距离处D 无法确定4、量子化学中,计算分子能量常用的方法是()A 半经验方法B 从头算方法C 密度泛函理论D 以上都是5、下列哪种化学键具有明显的量子力学特征()A 离子键B 共价键C 金属键D 氢键6、在量子化学中,分子轨道是由原子轨道线性组合而成,这一原理被称为()A 杂化轨道理论B 价键理论C 分子轨道理论D 晶体场理论二、填空题(每题 5 分,共 30 分)1、量子力学的基本假设包括波函数假设、算符假设、测量假设、全同性原理和__________________ 。
2、氢原子的薛定谔方程在球坐标下的解中,径向波函数 R(r) 与__________________ 有关。
3、多电子原子的电子排布遵循的原则有能量最低原理、泡利不相容原理和__________________ 。
4、分子的偶极矩是衡量分子__________________ 的物理量。
5、密度泛函理论的核心思想是将体系的能量表示为__________________ 的泛函。
6、量子化学计算中,常用的基组有 STO-3G、6-31G 等,其中 6-31G 表示的是__________________ 。
三、简答题(每题 10 分,共 20 分)1、简述量子化学中 HartreeFock 方法的基本思想。
2、解释为什么分子的振动光谱通常具有一系列的吸收峰,而不是单一的吸收峰。
四、计算题(共 20 分)已知氢原子处于某一激发态的波函数为:ψ =1/√8π a₀³(r/a₀) exp(r/2a₀) ,其中 a₀为玻尔半径。
基础量子化学练习定稿版

基础量子化学练习精编W O R D版IBM system office room 【A0816H-A0912AAAHH-GX8Q8-GNTHHJ8】2010基础量子化学练习(1)一、 判断正误( )1、 一个态函数总是等于时间的函数乘以坐标的函数。
( )2、 态函数总是Hamiltonian 算符的本征函数。
( )3、 Hamiltonian 算符的本征函数的任意线性组合是Hamiltonian 算符的本征函数。
( )4、 如果态函数不是算符ˆA的本征函数,则性质A 的一次测量可给出一个不是ˆA 的本征值的值。
( )5、 几率密度与时间无关。
( )6、 如果两个算符具有共同的本征函数,那么这两个算符可对易。
( )7、 算符ˆx 与d i dx -可对易。
( )8、 氢原子Hamiltonian 算符的束缚态的本征函数构成完备集。
( )9、 厄米算符的本征函数是正交的。
( )10、 描述电子轨道运动的波函数必须是奇函数。
二、已知:2ˆˆˆ,A d dx B x ==,计算2ˆˆˆˆ,()A B A B ⎡⎤+⎣⎦及 三、已知:11223344ˆˆˆˆ,,,,A a A b A a A d ϕϕϕϕϕϕϕϕ====如果任意状态可以表示为12343253,ψϕϕϕϕ=+++那么当我们对该状态进行测量时,获得a 和d 的几率各是多少?求任意状态 的性质A 的平均值。
2010基础量子化学练习(2)一、 判断正误( )11、 算符ˆˆˆ,,A B C 满足ˆˆˆˆ,0,,0A B A C ⎡⎤⎡⎤==⎣⎦⎣⎦,则三个算符存在共同的本征函数集。
( )12、 不能对易的算符不可能具有共同的本征函数。
( )13、 当对本征态的性质A 进行测量时,能够得到的唯一仅有的值是算符ˆA的本征值。
( )14、 如果一个算符的平方等于单位算符,那么这个算符的本征值等于+1或者-1。
( )15、 所有品优的奇函数和偶函数都是宇称算符的本征函数。
量子化学习题解(仅供参考)

ˆ ÂB ˆ BÂ= ˆ ˆ ÂB) ˆ = [B, ˆ Â] 证明:(1) [Â, B]= (BÂ (2)[Âm,Ân]= ÂmÂnÂnÂm= Âm+nÂm+n=0 ˆ BÂ ˆ 2 ˆ Â2B (3) [Â2, B]= ˆ ÂBÂ+ ˆ ˆ BÂ ˆ 2= Â2B ˆ BÂ ˆ 2 ˆ ˆ ˆ BÂ)+ ˆ ˆ BÂ)Â ˆ ÂBÂ Â[Â, B]+[Â, B]Â= Â(ÂB (ÂB = Â2B ˆ Â[Â, B]+[Â, ˆ ˆ [Â2, B]= B]Â ˆ Ĉ]]+ [B, ˆ [Ĉ, Â]]+ [Ĉ, [Â, B]] ˆ (4) [Â, [B, ˆ ĈĈB)]+[ ˆ ˆ (ĈÂÂĈ)]+ [Ĉ, (ÂB ˆ BÂ)] ˆ =[Â, (B B, ˆ ĈÂĈB ˆ B ˆ ĈÂ+ĈBÂ+ ˆ B ˆ ĈÂBÂ ˆ Ĉ ĈÂB+ ˆ ÂĈB+ ˆ ĈÂB ˆ ĈBÂ ˆ ÂB ˆ Ĉ+BÂ ˆ Ĉ=0 = ÂB ˆ p ˆ 2 2m V ( x ) , 分别计算(1)当 V(x)=V(常数), (2)当 V(x)=kx2/2, (3)当 V(x) V(r)=e2/40r 1.9 H
(12) [d/dx, d2/dx2]=0
1.7 如果 Â 是线性算符,b,c 为常数,f, g 为任意函数,证明 Â(bf+cg)= bÂf + cÂg;证明若 Â(bf+cg)= bÂf + cÂg,则 Â 一定是线性算符。 1)证明: Â 是线性算符 Â(bf+cg)= Â(bf) + Â(cg) = bÂf + cÂg 2)证明: Â(bf+cg)= bÂf + cÂg b,c 为常数
量子化学卷一答案

�B×(10) (10)×B�
B�A� B�n−1 − B�nA� = (n − 1)B�n−1 A�B�n − B�n−1A�B� = (n − 1)B�n−1
(13) (14)
(11)+(12)+(13)+(14)
A�B�n − B�nA� = nB�n−1
故得证
4. 已知f(x)和f ’(x)分别属于H�算符的不同本征值 E 和 E’的本征函数,那么它们的线性组合
4/4
dr
=
3 2
a0
7. 试计算一个立方势箱(边长为 a)中,粒子质量为 m,在能量由0~16h2/8ma2之间有多少
个能级?各自相应的能级简并度如何?在这个能量范围内有多少种状态?
解:对于三维立方势箱中粒子,其薛定谔方程为: ℏ2 ∂2 ∂2 ∂2
− 2m �∂x2 + ∂y2 + ∂z2� ψ = Eψ
(5)+(6)+(7)+(8)
A�B�3 − B�3A� = 3B�2
(9)
下面我们用数学归纳法证明最后一个式子
当 N=1 时,A�B� − B�A� = 1成立
当 N=2 时,A�B�2 − B�2A� = 2B�成立
设当 N=n-1 时,该式子成立,则A�B�n−1 − B�n−1A� = (n − 1)B�n−2
b
a
∫02
sin2
kπx a
dx
+
2 a
b
∫LL 2
sin2
kπx a
dx
=0
���������������(���������1)
=
���������������(���������0)
应化所量子化学考试题库及其答案详解

Principles Of Quantum Chemistry——Kwong.S.T名词解释1.测不准关系:()()41M L 22≥∆⋅∆{∫ψ*i[L ,M ]ψdx}22.酉矩阵:S +=S -13.厄米算符:算符L 满足∫u 1*(x)L u 2(x)dx=∫u 2(x)L *u 1*(x)dx,其中u 1(x)和u 2(x)是任意两个平方可积函数,积分遍于自变量全部区域。
则称L 是厄米算符。
4.等价表示:矩阵群M 和M’所包含的每一个对应矩阵之间只差一个同样的相似变换,就说M’和M 是等价表示。
5.可约表示:如果一个矩阵表示可以表示成子矩阵的直和,那么这个矩阵表示是可约表示。
6.不可约表示特征标:不可约表示矩阵群的对角元素之和称为这个不可约表示的特征标。
7.投影算符:P R R hl k j Rj j k*∑Γ=λλ)()()(,其中R 为群元素,*Γk j R λ)()(是第j 个不可约表示操作R 的第λ行第k 列的矩阵元,l j 是第j 个不可约表示的维数,h 为群的阶。
8.轨道近似:认为各个电子的运动是彼此独立的,每个电子都在核与其它电子所形成的稳定的平均场中运动,从而每个电子的状态可以用一个单电子波函数来描写。
9.定域分子轨道:认为成键电子只是集中在相邻两原子间的键轴区域内。
10.正则分子轨道:由HFR 方程解出的分子轨道。
11.Slater 行列式:描述多电子体系满足保里原理的波函数。
12.ab initio :严格的按HFR 方程进行计算称为从头计算。
13.NDO :假设原子轨道在空间任何地方都不重叠。
DO :对所有不同原子轨道的乘积都采用忽略微分重叠近似。
15.NDDO :对属于同一原子各对轨道重叠的排斥积分全部予以保留。
16.INDO :只保留单中心积分中同一原子对各轨道的微分重叠。
17.EHMO :整个分子不在一个平面上,则σ,π分离就不可能,于是就必须对分子中所有原子的所有价电子进行计算,若这种计算不是基于自洽场分子轨道理论,就称之为推广的休克尔分子轨道法。
量子化学试题及答案

6-31G*=6-31G(d)6:代表每个内层轨道由六个高斯型基函数拟合而成;价层轨道劈裂成两个Salter型基函数,内层轨道不发生劈裂,其中一个Salter型基函数由一个Gauss型基函数拟合而成,另一个Salter型基函数由一个Gauss型基函数拟合而成;d:表示要对出氢以外的原子都要加d轨道Salter型基函数:2*4+(1+2+2*3+6)*2=38Gauss型基函数:4*4+(6+4+4*3+1*6)*2=722、解:第一种方法:CH,1, 1.08290068H,1, 1.08290068,2, 109.47122063H,1, 1.08290068,2, 109.47122063,3,120.0,0H,1, 1.08290068,2, 109.47122063,3,-120.0,0第二种方法:CH 1 B1H 1 B2 2 A1H 1 B3 2 A2 3 D1 0H 1 B4 2 A3 3 D2 0B1 1.08290068B2 1.08290068B3 1.08290068B4 1.08290068A1 109.47122063A2 109.47122063A3 109.47122063D1 120.00000000D2 -120.000000003、解:在分子势能面上有五类极值点,分别如下:整体极小点、局部极小点、整体极大点、局部极大点及鞍点。
整体极小点:整个势能面上的最低点,代表了能量最低也就是最稳定的结构;局部极小点:势能面某个区域内的最低点,代表了局部区域内能量最低的点;整体极大点:整个势能面上的最高点,代表了能量最高的点;局部极大点:势能面某个区域内的最高点,代表了局部区域内的能量最高的点;鞍点:在一个方向上是极小点,其他方向上都是极大点,代表了体系的过渡态。
判断某一极值点是否为过度态:首先,是否有且只有一个虚频(数值为负值,足够大,一般上百);其次,看虚频的震动模式是不是朝着反应物和产物的方向震动;再次,进行IRC计算,看看是不是总想了反应物和产物。
结构化学 第一章 量子化学基础 习题

1029
y y y 设 体 系 处 在 状 态 =c1 211+ c2 210 中 , 角 动 量 M2 和 Mz 有 无 定 值 。其值为多少?若无 ,
则求其平均值。
1030
h¶
试 求 动 量 算 符 pˆ x=
的 本 征 函 数 (不 需 归 一 化 )。
i2p ¶x
1031
y 下 列 说 法 对 否 :” =cos x, px 有确 定 值 , p2x 没 有 确 定 值,只有平均值 。” ---------- ( )
(A) 16.5 × 10 -24? J (B) 9.5
× 10 -7 J (C) 1.9
× 10 (E) 1.75 × 10 -50? J
1039 一个在一维势箱中运动的粒子,
(1) 其 能 量 随 着 量 子 数 n 的 增 大 :------------------------ ( ) (A) 越 来 越 小 (B) 越 来 越 大 (C) 不变 (2) 其 能 级 差 En+1-En 随 着 势 箱 长 度 的 增大 : -------------------( ) (A) 越 来 越 小 (B) 越 来 越 大 (C) 不变
(A)
Aˆ U=λU, λ=常数
(B)
Bˆ U=U*
(C)
Cˆ U=U2
(D)
Dˆ U = dU
dx
(E)
Eˆ U=1/ U
1026 物 理 量 xp y- ypx
1027
_____ 。
某 粒 子 的 运 动 状 态 可 用波 函 数y =Ne-ix 来表 示 , 求 其 动 量 算符 pˆ x 的 本 征 值 。
1013
测 不 准 原 理 的 另一 种 形 式 为 Δ E·Δt≥h/2 π。当一个电子从 高能级向低能级跃迁 时,
量子化学习题附答案_

Py基础量子化学习题1.为什么微观粒子的运动状态要使用量子力学来描述,而不能用经典力学来描述?答:以牛顿三大定律为中心内容的经典力学适用于宏观物体的机械运动,当经典力学的应用范围推广到高速运动和小线度范围时,实验表明经典力学遇到了不可克服的困难。
对微观体系的研究导致了量子力学的诞生。
因为许多微观粒子都具有波粒二象性。
而在观察之前,我们得不到一个粒子的确切位置,它是一道弥散的波。
可以通过波函数来表示它们在每一区域出现的概率。
所以,描述描述微观粒子的运动状态要用量子力学。
2. 原子 A 和原子 B 在 y 轴上,指出 A 原子的 Px, Py, Pz 原子轨道与 B 原子的 dxy, dyz, dz 2 原子轨道间分别可形成什么分子轨道?①原子 A 的 Px 原子轨道与原子 B 的任意原子轨道无法形成分子轨道②原子 A 的 Py 原子轨道与原子 B 的 dyz 原子轨道形成π分子轨道(如图 1)图(1)③原子 A 的 Pz 原子轨道与原子 B 的 dyz 原子轨道形成σ 型分子轨道(如图 2)图(2)3. 原子 A 和原子 B 在 y 轴上,指出 A 原子的 px, py, pz 原子轨道与 B 原子的 dxy, dyz, dz2 原子轨道间分别可形成什么分子轨道?①原子 A 的 Px 原子轨道与原子 B 的 dxy 原子轨道形成π型分子轨道(如图 3)。
②原子 A 的 Py 原子轨道与原子 B 的任意原子轨道都无法形成分子轨道。
③原子 A 的 Pz 原子轨道与原子 B 的 dyz 原子轨道形成σ 型分子轨道(如图 4)。
∑ ∑ 10∑ ∑ 10 R )0 14. 写出任意一个原子的能量算符。
写出 Na+和 F-的薛定谔方程算符表达式。
ˆ h 22e 2H 原子的能量算符: H = -8π 2μ∇ - 4πε rNa +的薛定谔方程H ˆ = - h 10102i 11e 2+ ∑∑ e 8π 2 m i =1 i =1 4πε 0 r ii =1 i > j 4πε 0 r ijH ˆ ψ = E ψF -的薛定谔方程: H ˆ = - h10 10 2 i 9e 2 + ∑∑ e 8π 2 m i =1 i =1 4πε0 r ii =1 i ≠ j 4πε0 r ijH ˆ ψ = E ψ5. 写出任意一个分子的能量算符。
量子化学试题及答案

6-31G*=6-31G(d)6:代表每个内层轨道由六个高斯型基函数拟合而成;价层轨道劈裂成两个Salter型基函数,内层轨道不发生劈裂,其中一个Salter型基函数由一个Gauss型基函数拟合而成,另一个Salter型基函数由一个Gauss型基函数拟合而成;d:表示要对出氢以外的原子都要加d轨道Salter型基函数:2*4+(1+2+2*3+6)*2=38Gauss型基函数:4*4+(6+4+4*3+1*6)*2=722、解:第一种方法:CH,1, 1.08290068H,1, 1.08290068,2, 109.47122063H,1, 1.08290068,2, 109.47122063,3,120.0,0H,1, 1.08290068,2, 109.47122063,3,-120.0,0第二种方法:CH 1 B1H 1 B2 2 A1H 1 B3 2 A2 3 D1 0H 1 B4 2 A3 3 D2 0B1 1.08290068B2 1.08290068B3 1.08290068B4 1.08290068A1 109.47122063A2 109.47122063A3 109.47122063D1 120.00000000D2 -120.000000003、解:在分子势能面上有五类极值点,分别如下:整体极小点、局部极小点、整体极大点、局部极大点及鞍点。
整体极小点:整个势能面上的最低点,代表了能量最低也就是最稳定的结构;局部极小点:势能面某个区域内的最低点,代表了局部区域内能量最低的点;整体极大点:整个势能面上的最高点,代表了能量最高的点;局部极大点:势能面某个区域内的最高点,代表了局部区域内的能量最高的点;鞍点:在一个方向上是极小点,其他方向上都是极大点,代表了体系的过渡态。
判断某一极值点是否为过度态:首先,是否有且只有一个虚频(数值为负值,足够大,一般上百);其次,看虚频的震动模式是不是朝着反应物和产物的方向震动;再次,进行IRC计算,看看是不是总想了反应物和产物。
《量子化学基础》习题课
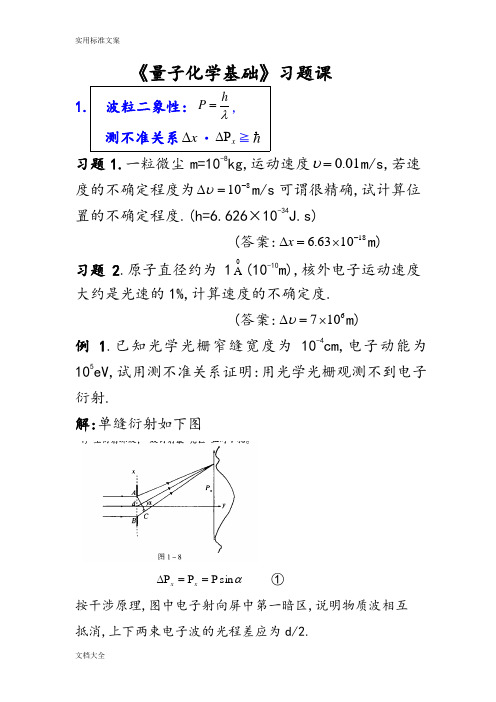
《量子化学基础》习题课 1. 波粒二象性: λhP =,测不准关系x ∆·x ∆P ≧习题1.一粒微尘m=10-8kg,运动速度01.0=υm/s,若速度的不确定程度为810-=∆υm/s 可谓很精确,试计算位置的不确定程度.(h=6.626×10-34J.s)(答案:181063.6-⨯=∆x m)习题2.原子直径约为10A (10-10m),核外电子运动速度大约是光速的1%,计算速度的不确定度.(答案:6107⨯=∆υm)例1.已知光学光栅窄缝宽度为10-4cm,电子动能为105eV,试用测不准关系证明:用光学光栅观测不到电子衍射.解:单缝衍射如下图αsin P =P =∆P x x ①按干涉原理,图中电子射向屏中第一暗区,说明物质波相互抵消,上下两束电子波的光程差应为d/2.λαα=⋅∆=⋅=sin sin x d BC ②这里410-=∆=x d cm,meV h mE h h 22==P =λ (=m 9.11×10-31kg ;=e 1.602×10-19C) 51025.1225.12==V λ=3.87×10-12m 661210101087.3sin ---=⨯==m m d λα 0≈α 证毕. 习题3.计算动能为3000eV 的电子的de Brogle 波长(1eV=1.602×10-19J, V 3000C10602.1J 10602.130001919=⨯⨯⨯=--V ) (答案: 0A 2237.0300025.1225.12===V λ.) 2 .一维势箱: 2228ml h n E =,),2,1( =nx ln C x πsin )(2=ψ 习题4.计算箱宽为5×10-10m 的一维势箱中粒子n=1、2时的能量.及粒子从n=2跃迁到n=1时辐射的波长. (答案:E 1=2.41×10-19J,E 2=9.64×10-19J191023.7-⨯=∆E J 71075.2-⨯=λm) 习题 5.可将原子中的电子粗略的模拟为一维箱中粒子,箱的宽度为原子的尺度.计算在长度10A 的箱中电子两个最低能级之差(eV)和在此两能级间跃迁的光子波长(cm). (答案:21013.1⨯=∆E eV, 8101.1-⨯=λm)例2. 丁二烯(H 2C=CH-CH=CH 2)4个π电子(P z 电子),可以近似地看作长度为大Π键键长(已知:双键长035.1A 、单键长054.1A )的一维势箱中的4个粒子的运动.体系的基态向第一激发态的跃迁为:_______1_______2___________3___________4↑↓=↑↓===n n n n 1_______2________3________4___________=↑↓=↑=↑=n n n n求:体系基态向第一激发态的跃迁能(cm -1).解.箱长:m A A l 10001078.554.1235.12-⨯=⨯+⨯=.)23(888222222222223-=-=∆l m h l m h n l m h n E e e e 21031234)1078.5(1011.985)10626.6(m kg s J ---⨯⨯⨯⨯⨯⋅⨯=J 191002.9-⨯=.14834191054.4/10310626.61002.9----⨯=⨯⨯⋅⨯⨯=∆=cm s m s J J hc E ν 例3.有一个量子数为n 在长度为l 的一维箱中运动的粒子(1)计算在箱的左端41区内找到粒子的几率.(2)n 为何值时该几率最大?(3)计算当n →∞时该几率的极限?解: 2sin 2141)sin 2(2410πππn n dx x l n l P -==⎰⎪⎩⎪⎨⎧==-==8,6,4,2,015,11,7,3,113,9,5,1,12sin n n n n 当当当π 即,n=3时,P 最大.π6141max +=P . 41)2sin 2141(lim =-∞→ππn n n (该式说明:当n →∞时量子力学问题还原为经典(力学)问题,因为经典力学处理一维箱问题在左端1/4处的几率正是1/4.)习题6.有一质量为1g 的小球,在长为10cm 的一维箱中运动小球速度0.1cm/s,试求小球的量子数n 是多少 ? (答案:n=3.018×1026) 3. 一位谐振子:m k πν21=)21(+=υνh E ( 3,2,1,0=υ) 习题7.HI 近红外光谱的基本振动频率22300=-ν.1cm -1,求HI 键的弹力常数k . (答案:k =2.93×105 D.cm -1) 习题8.已知H 2和Cl 2的基本振动频率分别为24.4395)(20=H νcm -1,9.564)(20=Cl νcm -1,求它们的零点能. (答案:E 0(H 2)=6.28kg/mol, E 0(Cl 2)=0.807kg/mol) 例 4.利用简谐振动公式:)sin()(φω+=t C t x ,证明一维谐振子的总能量是常数:221kC E =解:)(sin 21)(cos 2122222φωφωω+++=+=t kC t C m V T E m k =2ω ,带入上式,得: 221kC E =. 习题9.若H 35Cl 基本振动频率13010667.8⨯=νs -1,试求H 37Cl 的基本振动频率,设它们键的力常数相同.(答案:8.660×1013s -1) 4. 刚性转子:)1(22+=l l IE ( 3,2,1,0=l ) )1(2)(+=l B l ν (式中Ic hB 28π=) 例5.由HCl 的远红外光谱数据得知HCl 的转动能级相邻谱线间距为20.74cm -1,求HCl 分子的键长r.解: c r hIc h B 222442μππν===∆24231063.11002.6)45.35008.1(45.35008.1-⨯=⨯+⨯=+⋅=Cl H Cl H m m m m μg/mol 211024227212)2/74.20(1031063.1)1416.3(810626.6)8(][⨯⨯⨯⨯⨯⨯==--cB h r μπ =1.287×10-8cm=1.2870A习题10.从CN 的微波谱知道一系列谱线的间距皆为3.7978cm -1,求该分子的核间距r.(答案:r=1.172×10-10m)习题11.已知CN +的键长是1.290A ,指出CN +微波谱的第一到第四条线的位置.(答案:3.134cm -1,6.268cm -1,9.402cm -1,12.536cm -1 5.算符:),,(t q P F ∧∧其中q i ∂∂-=P ∧与本征函数:∧A )(x f =k )(x f习题12.写出下列物理量的算符:(1)动量P,(2)动量3y p ,(3)x y yp xp -,(4)动能T( 答案(1))(z k y j x i i P ∂∂+∂∂+∂∂-=∧,(2)3333y i P y ∂∂=∧(3))()(x y y x i p y p x L x y z ∂∂-∂∂-=-=∧∧∧ (4) )(22222222z y x m T ∂∂+∂∂+∂∂-=∧) 13.计算算符222kx dx d A -=∧的本征函数2x e α-的本征值(答案:22)24()(222222x x e kx x e kx dx d αααα----=- )14.利用[∧D ,∧x ]=1,(1)计算2)(x D +∧,(2)))((x D x D -+∧∧ (答案:(1)2)(x D +∧1222+++=∧x D x D (2)1))((22--=-+∧∧∧x D x D x D )15.三维箱中粒子的波函数是下列那些算符的本征函数(1)∧x p ,(2)∧2x p ,(3)∧2z p ,(4)∧2x .(答案(2),(3) )6.对易计算以下对易恒等式可方便对易运算:①.],[],[∧∧∧∧-=A B B A②.0],[=∧∧n A A (n=1,2,3…)③.],[],[],[∧∧∧∧∧∧==B A k kB A B kA④],[],[],[∧∧∧∧∧∧∧+=+C A B A C B A⑤ ],[],[],[∧∧∧∧∧∧∧∧∧+=C A B C B A C B A 例6.计算对易子:],[∧x P x ,],[2∧x P x ,],[∧H x ,],[∧∧H P x .解: i i x x i x x i x i x P x x =-=∂∂-=∂∂=∂∂=∧],[],[],[],[ ],[],[],[2∧∧∧∧∧+=x x x x x P x P P P x P xx i i x i i ∂∂⋅+∂∂⋅= x ∂∂=22)](21,[],[)],,(,[],[222∧∧∧∧∧∧++==+=z y x P P P m x T x z y x V T x H x ∧∧=∂∂==x x P mi x m P x m 22],[21 ],[)](,[21],[222V P P P P P m H P x z y x x x ∧∧∧∧∧∧∧+++= x V i V x i V P x ∂∂-=∂∂-==∧],[],[(式中x V f x f V x Vf f x V V x f V x ∂∂=∂∂-∂∂=∂∂-∂∂=∂∂)()(],[)16.计算:],[222c bx ax dx d ++ (答案: ],[222c bx ax dx d ++=dxd b ax a )24(2++. ) 例7.证明:222)(〉〈-〉〈=∆A A A解: 〉〉〈-〈≡≡∆∧222)()(A A A A στψψd A A ⎰〉〈-=∧*2)( τψψτψψτψψτψψd A d A A d A A d A ⎰⎰⎰⎰〉〈+〉〈-〉〈-=*∧*∧*∧*22τψψτψψτψψd A d A A d A A A ⎰⎰⎰*∧*∧*〉〈+〉〈-〉〈-〉〈=22 2222〉〈+〉〈-〉〈-〉〈=A A A A 22〉〈-〉〈=A A 证毕 7. H 原子薛定谔方程的解222204118n R n h e E n -=-=εμ (n=1,2,…),其中22048h e R εμ-=. )()()(,,,θϕθϕψm l l n m l n Y r R r =)()()(,,ϕθm m l l n r R ΦΘ= ϕθim ml na Zr i l l n i i e P e a Zr c C )(cos })({0101--+-=∑= 氢原子波函数受控于三个量子数(m l n ,,)∞=,,2,1 n1,,2,1,0-=n ll m ±±±=,,2,1,0习题17.下列函数(1)2P z ,(2)2P x ,(3)2P 1分别是算符∧H 、∧2L 、∧z L 中哪个的本征函数. (答案:(1)、(3)全是,(2)是∧H 、∧2L 的本征函数) 习题18.对H 原子P 态,测量L Z 的可能值为h 、0、-h,问在下列函数中测量L Z 得到各可能值的几率.(1)Pz 2ψ,(2) Px 2ψ,(3) 12P ψ(答案:(1) 121211210200-⋅+⋅+=ψψψψPz得0值几率为1,得h 、-h 几率为0. (2) 121211*********-++⋅=ψψψψPx 得h 、-h 几率各为21,得0值几率为0.(3) 12P ψ=211ψ,得h 几率为1,其余为0) 例8.已知H 原子)(ϕΦ方程复数解:ϕπϕim m e 21)(=Φ,(1)验证)(ϕm Φ是归一化的.(2)证明)(ϕm Φ是算符ϕ∂∂-=∧ i L z 的本征函数.而)(ϕm Φ线性组合的实函数: ⎪⎪⎩⎪⎪⎨⎧=Φ=Φϕπϕϕπϕm m m msin 1)(cos 1)('`不是∧z L 的本征函数.解:(1)121212020202===ΦΦ=Φ⎰⎰⎰⎰-*ππϕϕπϕπϕπϕϕd d e e d d im im (2) )(21)(ϕπϕϕϕm im m z m e i L Φ±=∂∂-=Φ±∧而 ϕπϕπϕϕm m i m i L m z sin cos 1)( =∂∂-=Φ∧不构成本征方程: )()(ϕϕm m z c L Φ≠Φ∧.例如n=2,l =1的H 原子波函数是p 轨道,有三个状态(m=1,0,-1),其复函数形式为:θθϕψcos )()(0,1,2r r f r = (即:z p p 也是0)ϕθθϕψi e r r f r ±±=sin )()('1,1,2 (即:11-p p 和)其中复函数z p p 等于实函数0,复函数11-p p 和线性组合为实函数y x p p ,,即ϕθθϕϕcos sin )()(sin )(11r R N e e r R N p i i x =+=- ϕθθϕϕsin sin )()(sin )(11r R N e e r R N p i i y =-=- 因此,z p p 和0都是∧z L 的本征函数. 11-p p 和也是∧z L 的本征函数.只有y x p p ,不是∧z L 的本征函数.P 轨道的实函数与复函数形式都是体系的状态函数,但表示图形不一样:例9.对H 原子基态,(1)求2倍玻耳半径之外的电子几率.(2)玻耳半径之内的电子几率. 解:H 原子基态,n=1,0=l ,m=0301,,,1)()()(a r s ml l n m l n eaY r R r -=→=πψθϕθϕψ(1)设P 2为02a 之外的电子几率 ϕθθπτψππd drd re a d P a r a s sin 1122200203021200-⎰⎰⎰⎰==-⎰⎰⎰-=ππϕθθπ2022023sin 10d d dr e r a a r adr e r a a ra 002202304-⎰=ππ利用积分公式][0203020022302)82422(41a a r ara a r ea P ---=--)22(3222b b x b x e dx e x bx bx +-=⎰]4)44448([430303030430a a a a e a +---=- 4131e -= 即: 24.01342==e P(2)设1P 是玻耳半径0a 之内的电子几率,则dr e r a P a r a 02023014-⎰=][00302002230)82422(4a a r a ra a r e a ---=-]4)44242([430303030230a a a a e a +---=-32.0512=-=e例10.已知类氢离子基态波函数 03031a zr s ea z-=πψ,(1) 求半径r 的平均值.(2)r 的最可几值.解:(1)ϕθθπτψψππd drd r ea zd r r a zr ssin 32002030310-∞*⎰⎰⎰⎰==〉〈dr er a za zr 02033034-∞⎰⋅=ππ利用广义积分公式:10!+∞-=⎰n qxn qn dx ex (0,1>->q n )z a a z a z dr e r a z r a zr23)2(!344040303023330=⋅==〉〈⎰∞-(2)径向分布函数: 0223032214)(a zr ser a z r r R D -=⋅=求最可几半径就是求最大径向分布函数时r,0)22(4)(40202230322303=-==---a zr a zr a zr ea z r rea zer dr d a z dr dD020=-r r a z 即, z a r 0=为最可几半径.。
量子化学试题及答案

量子化学试题及答案一、选择题(每题2分,共10分)1. 量子化学中,描述电子运动状态的基本方程是:A. 薛定谔方程B. 牛顿运动方程C. 麦克斯韦方程D. 热力学第一定律答案:A2. 以下哪个不是量子化学中的量子数?A. 主量子数B. 角量子数C. 磁量子数D. 动量量子数答案:D3. 根据泡利不相容原理,一个原子轨道最多可以容纳:A. 1个电子B. 2个电子C. 3个电子D. 4个电子答案:B4. 量子化学中,分子轨道理论认为分子轨道是由:A. 原子轨道的叠加B. 原子轨道的差分C. 原子轨道的线性组合D. 原子轨道的非线性组合答案:C5. 以下哪个不是量子化学中的波函数?A. 波恩-奥本海默近似B. 哈密顿算符C. 原子轨道D. 分子轨道答案:B二、填空题(每题2分,共10分)1. 量子化学中,电子的波动性可以通过______方程来描述。
答案:薛定谔2. 根据量子化学理论,原子轨道的能级是由______量子数决定的。
答案:主3. 量子化学中,分子的电子云分布可以通过______轨道理论来分析。
答案:分子轨道4. 量子化学中,电子的自旋量子数可以取值为______。
答案:±1/25. 量子化学中,原子轨道的径向分布函数通常用______来表示。
答案:R(r)三、简答题(每题5分,共20分)1. 简述量子化学中波函数的物理意义。
答案:波函数是量子化学中描述电子状态的数学函数,它包含了电子在空间中出现的概率分布信息。
2. 解释量子化学中量子数的作用。
答案:量子数是量子化学中用来描述电子在原子轨道中运动状态的一组整数或半整数,包括主量子数、角量子数、磁量子数和自旋量子数,它们决定了电子的能量、角动量和自旋状态。
3. 描述量子化学中分子轨道理论的基本原理。
答案:分子轨道理论是基于量子力学的基本原理,认为分子轨道是由原子轨道的线性组合形成的,分子轨道的形成可以解释分子的化学性质和稳定性。
4. 量子化学中,如何理解电子的波粒二象性?答案:电子的波粒二象性是指电子既可以表现出粒子的性质,如在原子中占据特定的轨道,也可以表现出波动的性质,如干涉和衍射现象。
量子化学课程习题及标准答案
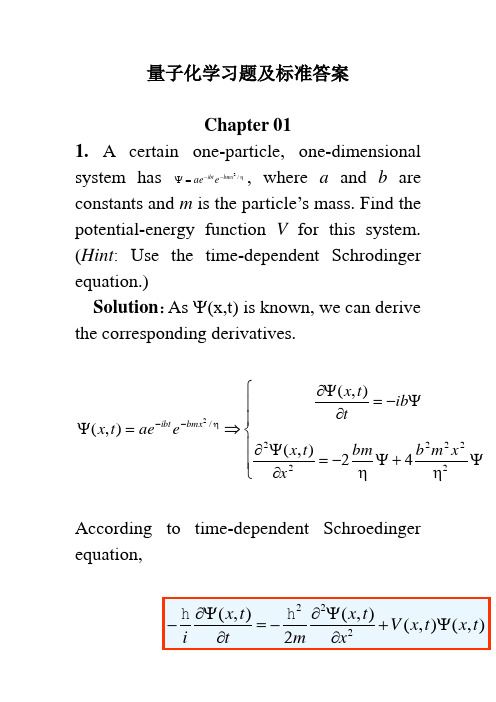
量子化学习题及标准答案Chapter 011. A certain one-particle, one-dimensional system has η/2bmx ibt e ae --=ψ, where a and b are constants and m is the particle ’s mass. Find the potential-energy function V for this system. (Hint : Use the time-dependent Schrodinger equation.)Solution :As ψ(x,t) is known, we can derive the corresponding derivatives.⎪⎪⎩⎪⎪⎨⎧ψ+ψ-=∂ψ∂ψ-=∂ψ∂⇒=ψ--222222/42),(),(),(2ηηηx m b bm xt x ib t t x e ae t x bmx ibtAccording to time-dependent Schroedinger equation,substituting into the derivatives, we get222),(mx b t x V =2. At a certain instant of time, a one-particle, one-dimensional system has bx xe b /||2/13)/2(-=ψ, where b = 3.000 nm. If a measurement of x is made at this time in the system, find the probability that the result (a) lies between 0.9000 nm and 0.9001 nm (treat this interval as infinitesimal); (b) lies between 0 and 2 nm (use the table of integrals, if necessary). (c) For what value of x is the probability density a minimum? (There is no need to use calculus to answer this.) (d) Verify that ψ is normalized.Solution :a) The probability of finding an particle in a space between x and x+dx is given by6/223210*29.32--==ψ=dx e x b dx P b x b) 0753.02910*20/223==⎰--dx e x bP b x c) Clearly, the minimum of probability density is at x=0, where the probability densityvanishes. d)4220/223/223/2232===ψ=⎰⎰⎰⎰+∞-+∞∞--+∞∞--+∞∞-dxe x b dx e x b dx e x b dx P b x b x b x3. A one-particle, one-dimensional system has the state function2222/4/16/4/12)/32)((cos )/2)((sin c x c x xe c at e c at --+=ψππ where a is a constant and c = 2.000 Å. If the particle ’s position is measured at t = 0, estimate the probability that the result will lie between 2.000 Å and 2.001 Å.Solution :when t=0, the wavefunction is simplified as441610*158.2)32(),(22--==ψc x xe c t x πChapter 021. Consider an electron in a one-dimensional box of length2.000Å with the left end of the box at x = 0. (a) Suppose we have one million of these systems, each in the n = 1 state, and we measure the x coordinate of the electron in each system. About how many times will the electron be found between 0.600 Å and 0.601 Å? Consider the interval to be infinitesimal. Hint: Check whether your calculator is set to degrees or radians. (b) Suppose we have a large number of these systems, each in the n =1 state, and we measure the x coordinate of the electron in each system and find the electron between 0.700 Å and 0.701 Å in 126 of the measurements. In about how many measurements will the electron be found between 1.000 Å and 1.001 Å?Solution: a) In a 1D box, the energy and wave-function of a micro-system are given by)sin(2,22222x ln l ml n E πψπ==η therefore, the probability density of finding the electron between 0.600 and 0.601 Å is65510*545.6)(sin 242⇒==-dx x ln l P πb) From the definition of probability, the probability of finding an electron between x and x+dx is given bydx x l n l P )(sin 22π= As the number of measurements of finding the electron between 0.700 and 0.701 Å is known, the number of system is1(sin 22*158712158712001.0)7.02*1(sin 2212612622=⇒===πP P N2. When a particle of mass 9.1*10-28 g in a certain one-dimensional box goes from the n = 5 level to the n = 2 level, it emits a photon of frequency 6.0*1014 s -1. Find the length of thebox.Solution.lml h n n ml n n E lowerup lowerup 36222222222110*26646.18)(2)(-=-=-=∆ηπ3. An electron in a stationary state of a one-dimensional box of length 0.300 nm emits a photon of frequency 5.05*1015 s -1. Find the initial and final quantum numbers for this transition.Solution:2,388)(2)(22222222222===-⇒=-=-=∆lower upper lower up lower up lower up n n n n hv ml h n n ml n n E ηπ4. For the particle in a one-dimensional box of length l , we could have put the coordinate origin at the center of the box. Find the wave functions and energy levels for this choice of origin.Solution: The wavefunction for a particle in a one-dimernsional box can be written as)2()2()(x mE BSin x mE ACos x ηη+=ψ If the coordinate origin is defined at the center of the box, the boundary conditions are given as2()22(0)(2()22(0)(22mE BSin l mE ACos x mE BSin l mE ACos x l x lx +⇒=-⇒==-=ηηηηψψ Combining Eq1 with Eq2, we get)4(,0)22()3(,0)22(Eq l mE BSin Eq l mE ACos ==ηη Eq3 leads to A=0, or )22(l mE Cos η=0. We willdiscuss both situations in the following section.If A=0, B must be non-zero number otherwise the wavefunction vanishes.2220)22(02mlh n E n l mE l mE Sin B π=⇒=⇒=⇒≠ηηIf A ≠08)12()21(220)22(00)22(0)22(0222mlh n E n l mE l mE Cos B l mE Sin l mE Cos A ψπ⇒+=⇒+=⇒==⇒≠⇒=⇒≠ηηηη5. For an electron in a certain rectangular well with a depth of 20.0 eV , the lowest energy lies 3.00 eV above the bottom of the well. Find the width of this well. Hint : Use tanθ = sin θ/cos θ Solution : For the particle in a certain rectangular well, the E fulfill with )2sin()2()2cos()(21010l mE V E l mE E E V ---=-ηη Substituting into the V and E, we get1010011110*64.22)7954.0(7954.022)(2)2()2()2(-----=⇒+-=⇒+-=⇒-=--==lowest l mEn l n l mE V E E E V l mE Tan l mE Cos l mE Sin ππηηηηηChapter 031. If Aˆf (x ) = 3x 2 f (x ) + 2xd f /dx , give an expression for Aˆ. Solution :Extracting f(x) from the known equation leads to the expression of Adx d x x A 23ˆ2+=2. (a) Show that (Aˆ+B ˆ)2 = (B ˆ+A ˆ)2 for any two operators. (b) Under what conditions is (Aˆ+B ˆ)2 equal to Aˆ2+2A ˆB ˆ+B ˆ2? Solution: a)2222ˆ()ˆˆ)(ˆˆ(ˆˆˆˆˆˆˆˆˆˆˆ)ˆˆ)(ˆˆ()ˆˆ(B A B A BA B A A B B A B B A A B A B A B A =++=+++=+++=++=+ b) B AA B A B B A A B A ˆˆ2ˆˆˆˆˆˆˆ)ˆˆ(2222++=+++=+If and only if A and B commute, (Aˆ+B ˆ)2 equals to Aˆ2+2A ˆB ˆ+B ˆ2 3. If Aˆ = d 2/dx 2 and B ˆ = x 2, find (a) A ˆB ˆx 3; (b) B ˆA ˆx 3; (c) A ˆB ˆf (x ); (d)B ˆA ˆf (x )Solution: a)3522320ˆˆxx dxd x B A == b)3322236ˆˆx x dxd x x A B == c))()(4)(2)]()(2[)]([)(ˆˆ2222222x f dxd x x f dx d x x f x f dxd x x xf dx d x f x dx d x f B A ++=+==d))()()(ˆˆ222222x f dxd x x f dx d x x f A B ==4. Classify these operators as linear or nonlinear: (a) 3x 2d 2/dx 2; (b) ( )2; (c) ∫ dx ; (d) exp; (e) ∑=n x 1.Solution:Linear operator is subject to the following condition.f A c cf Ag A f A g f A ˆ)(ˆˆˆ)(ˆ=+=+ a) Linearb) Nonlinear c) Linear d) Nonlinear e) Linear5. The Laplace transform operator Lˆ isdefined by⎰∞-=0)()(ˆdx x f e x f L px(a) Is Lˆ linear? (b) Evaluate Lˆ(1). (c)Evaluate Lˆe ax , assuming that p >a . Solution:a) L is a linear operator b)0,1)1(ˆ0>==⎰∞-p p dx e L px c)pdx e dx e e e L x a p ax px ax ===⎰⎰∞--∞-)(ˆ0)(06. We define the translation operator hT ˆ by hT ˆf (x ) = f (x + h ). (a) Is hT ˆ a linear operator? (b) Evaluate (2ˆ3ˆ121+-T T )x 2. Solution:a) The translation operator is linear operator b)2212212121(2ˆ3ˆ)2ˆ3ˆ(x x x T x T x T T +=+-=+-7. Evaluate the commutators (a) [xˆ,xp ˆ]; (b) [xˆ,2ˆxp ]; (c) [x ˆ,yp ˆ]; (d) [x ˆ, ),,(ˆz y x V ]; (e) [x ˆ,H ˆ]; (f) [z yx ˆˆˆ, 2ˆxp ]. Solution: a)ηηηi xx x x i x x i p xx =∂∂--∂∂-=∂∂-=)ˆ1ˆ(],ˆ[]ˆ,ˆ[b)xp i p p x p x p p xx x x x x x∂∂==+=222ˆ2)ˆ]ˆ,ˆ[]ˆ,ˆ[ˆ(]ˆ,ˆ[ηηc)0)ˆˆ(],ˆ[]ˆ,ˆ[=∂∂-∂∂-=∂∂-=yx y x i y x i p xy ηηd)0ˆ),,(ˆ),,(ˆˆ)],,(ˆ,ˆ[=-=x z y x V z y x V x z y x V xe)mxT x V T x H x ==+=21,ˆ[]ˆ,ˆ[]ˆˆ,ˆ[]ˆ,ˆ[f)xz y p z y xx∂∂=ˆˆ2]ˆ,ˆˆˆ[22ηChapter 041:The one-dimensional harmonic-oscillator is at its first excited state and its wavefunction is given as)21exp()()(2)(24/14/31x x x βπβψ-=please evaluate the expectation values(average values) of kinetic energy (T), potential energy (V) and the total energy.Answer: 1) First of all, check the normalization property of the wavefunction.2) Evaluate the expectation value of kinetic energy.3) Evaluate the expectation value ofpotential energy4) Total Energy = T + V2. The one-dimensional harmonic-oscillator Hamiltonian is2222ˆ22ˆˆxm v mp H xπ+= The raising and lowering operators for thisproblem are defined as]ˆ2ˆ[)2(1ˆ2/1x ivm p m A x π+=+, ]ˆ2ˆ[)2(1ˆ2/1x ivm p m A x π-=-Show thathv H A A 21ˆˆˆ-=-+,hv H A A 21ˆˆˆ+=+-, hv A A-=-+]ˆ,ˆ[ ++=A hv A H ˆ]ˆ,ˆ[, ---=A hv A Hˆ]ˆ,ˆ[ Show that +Aˆ and -A ˆ are indeed ladder operators and that the eigenvalues are spaced at intervals of hv . Since both the kinetic energy and the potential energy are nonnegative, we expect the energy eigenvalues to be nonnegative. Hence there must be a state of minimum energy. Operate on the wavefunction for this state first with -Aˆ and then with +Aˆ and show that the lowest energy eigenvalue ishv 21. Finally, conclude that hvn E )21(+=, n = 0, 1, 2, …Answer:1) Write down the definition of operatordx di px η-=ˆ2) Expand the operators in full form.]2[21]2[2122ˆ222222vmxi dx di mA vmxi dx d i mA mx v dx d m H πππ--=+-=+-=-+ηηη 3) Evaluate the corresponding combination ofoperators4242[21]22][2[21ˆ4222[21]2[2]2[2[21]2[21]22[ˆ21ˆ2122]4222[21]]2[2]2[[21]2[21]2[2121ˆ2122]42[21]4222[21]]2[2]2[[21]2[21]2[21322222222333222222332222222333222222222222222222222222222222222222222222222v dx d ix v dx d i mx v mxi v dxd m i m mx v dx d m vmxi dx d i mH A v dx d i mx v dx d ix v dx d i v dxd m i m vmxi dx d i mx v vmxi dx d i dxd m m vmxi dx d i mmx v dx d m A H hv H hv mx v dx d m x m v dxd vmx dx d x vm vm dx d m vmxi dxd i vmxi vmxi dx d i dx d i m vmxi dx d i mvmxi dx d i m A A hv H hv mx v dx d m x m v vm dxd m x m v dxd vmx dx d x vm vm dx d m vmxi dxd i vmxi vmxi dx d i dx d i m vmxi dx d i mvmxi dx d i m A A πππππππππππππππππππππππππππππππππππππ+---=+-+-=+---=+-++--=+-+-=+=++-=+-++-=+--+--=+---=-=-+-=+--=++---=--+---=--+-=+++--+ηηηηηηηηηηηηηηηηηηηηηηηηηηηηηηηηηηηηη+++=+-=+-=+-=-hvA vmxi m dx d i m hv vmxi m hv dxd i m hv mxi v dx d i v m H A A H ]22121[]221[]21[]42[21ˆˆ222ππππηηηηIn the same manner, we can get---=-hvA H A A H ˆˆ 4) Substituting the above communicators into the Schroeidnger equation, we getψψψψψψψψψψψψ------++++++-=-=-=+=+=+==A hv E hvA E A hvA H A A H A hv E hvA E A hvA H A A H E H)(]ˆ[ˆ)(]ˆ[ˆˆThis shows that +Aˆ and -A ˆ are indeed ladder operators and that the eigenvalues are spaced at intervals of hv .5) Suppose that ψ is the eigenfunction with the lowest eigenvalue. ψψlowest E H =ˆAccording to the definition of A_ operator, we haveψψ---=A hv E A H )(ˆAs ψ is the eigenfunction with the lowest eigenvalue, the above equation is fulfilled if and only if 0=-ψAOperating on the wave function for this statefirst with -A ˆ and then with +A ˆ leads to ψψψψhv H hv H A A 21ˆ]21ˆ[0=⇒-==-+Therefore, the lowest energy is 1/2 hv.Λ3,2,1,0,)(21ˆ=+=n hv n H ψψChapter 051. For the ground state of the one-dimensional harmonic oscillator, compute the standard deviations ∆x and ∆p x and check that the uncertainty principle is obeyed.Answer:1) The ground state wavefunction of the one-dimensional harmonic oscillator is given by2214141)(x e ααπψ--=2) The standard deviations ∆x and ∆p x are defined as222x x x -=∆2 2)2∆=∆-(p(p∆)pThe product of ∆x and ∆p is given by2422122ηηη==•=∆∆ααp xIt shows that the uncertainty principle is obeyed.2. (a) Show that the three commutationrelations [x Lˆ,y L ˆ] = z L i ˆη, [y L ˆ,z L ˆ] = x L i ˆη, [z L ˆ,x L ˆ] = y L i ˆη are equivalent to the single relation L L Lˆˆˆηi =⨯ (b) Find [2ˆx L,y L ˆ] Answer:1): zy x y x z x z y z y x y x x z z y x y y x z x x z y z z y y z x z z y x y z x y x z y x z y x z y x L i L L L i L L L i L L k L j L i L i k L L j L L i L L k L L L L j L L L L i L L L L i L L j L L i L L k L L j L L k L L k L j L i L k L j L i L L L k L j L i L L ηηηρρρηρρρρρρρρρρρρρρρρρρρρρ===⇒++=++=-+-+-=-++--=++⨯++=⨯++=],[],[],[)(],[],[],[)()()()()(ˆˆˆ2):)()()(],[],[],[2x z z x x z z x x y x y x x y x L L L L i L L i L i L L L L L L L L L +=+=+=ηηη3. Calculate the possible angles between L and the z axis for l = 2.Answer:The possible angles between L and the z axis are equivalent the angles between L and L z . Hence, the angles are given by:Lm Cos L z ==+=θηη6)12(2 ︒︒︒︒=7.144,10.114,00.90,91.65,26.35θ4. Complete this equation:m l m l z Y m Y L 333ˆη=Chapter 061. Explain why each of the following integrals must be zero, where the functions are hydrogenlike wave functions: (a) <2p 1|z L ˆ|3p -1>; (b) <3p 0|z L ˆ|3p 0>Answer:Both 3p -1 and 3p 0 are eigenfunctions of L z , with eigenvalues of -1 and 0, respectively. Therefore, the above integrals can be simplified asa) due to orthogonalization properties of eigenfunctions03|213ˆ21111=-=--p p p L p z b) 02. Use parity to find which of the following integrals must be zero: (a) <2s |x |2p x >; (b) <2s |x 2|2p x >; (c) <2p y |x |2p x >. The functions in these integrals are hydrogenlike wave functions.Answer:1) b) and c) must be zero.3. For a hydrogen atom in a p state, the possible outcomes of a measurement of L z are – ħ, 0, and ħ. For each of the following wave functions, give the probabilities of each of these three results: (a)z p 2ψ; (b) y p 2ψ; (c) 12p ψ. Then find <L z > for each of these three wave functions.Answer:a) 022p p z ψψ=, therefore, the probabilities are:0%, 100%, 0% )(2111222-+=p p p x ψψψ, the probabilities are 50%, 0%, 50%.12p ψ,the probabilities are 100%, 0%, 0%b) 0,0,14. A measurement yields 21/2ħ for the magnitude of a particle ’s orbital angular momentum. If L x is now measured, what are the possible outcomes?Answer:1): Since the wavefunction is the eigenfunction of L2, a measurement of the magnitude of the orbital angular momentum should be+L=LLηη(=⇒21)1,The possible outcomes when measure L x are-1, 0, 1Chapter 071. Which of the following operators are Hermitian: d /dx , i (d /dx ), 4d 2/dx 2, i (d 2/d x 2)?Answer :An operator in one-D space is Hermitian if⎰⎰=dx A dx A **)ˆ(ˆψψψψ a)⎰⎰⎰⎰-=-=-=∞∞-dx dx d dx dx d dx dx d dx dx d *****)(ψψψψψψψψψψ b)⎰⎰⎰⎰=-=-=∞∞-dx dx d i dx d i dx dx d i i dx dxd i ****)(ψψψψψψψψψψc)⎰⎰⎰⎰⎰=+-=-=-=∞∞-∞∞-dxdxddxddxddxdxddxddxddxddxddxdxdψψψψψψψψψψψψψ2*22****22*44 444 44This operator can be written as a product of 1D kinetic operator and a constant. Hence, it’s Hermitian.d) As the third operator is Hermitian, this operator is not Hermitian.2. If Aˆand Bˆare Hermitian operators, prove that their product AˆBˆis Hermitian if and only if Aˆand Bˆcommute. (b) If Aˆand Bˆare Hermitian, prove that 1/2(AˆBˆ+BˆAˆ) is Hermitian.(c) Is x p xˆˆHermitian? (d) Is 1/2(x p xˆˆ+x p xˆˆ)Hermitian?Answer:1)If operator A and B commute , we have⎰=-⇒=-⇒=0])ˆˆˆˆ[(0ˆˆˆˆˆˆˆˆˆ*τψψd A B B A A B B A A B B A⎰⎰⎰=⇒=-⇒τψψτψψτψψd A B d B A d A B B A***]ˆˆ[]ˆˆ[0])ˆˆˆˆ[(Operator A and B are Hermitian, we have⎰⎰⎰==⇒τψψτψψτψψd B A d B A d A B ˆˆ)ˆ()ˆ(]ˆˆ[***Therefore, when A and B commute, the following equation fulfills. Namely, AB is also Hermitian.⎰⎰=τψψτψψd B A d B A ˆˆ]ˆˆ[**2)]ˆˆˆˆ[21)]ˆˆˆˆ(21[***⎰⎰⎰+=+τψψτψψτψψd A B d B A d A B B A Operator A and B are Hermitian, we get⎰⎰⎰⎰⎰⎰⎰+=+⇒+=+=+τψψτψψτψψτψψτψψτψψτψψd A B B A d A B B A d B A A B d B A d A B d A B d B A *******])ˆˆˆˆ(21[)ˆˆˆˆ(21])ˆˆˆˆ(21[)ˆˆ()ˆˆ([21]ˆˆˆˆ[21The above equation shows that the operator 1/2[AB+BA] is Hermitian.c) xp x is not Hermitian since both x and px are Hermitian and do not commute.d) YesChapter 081. Apply the variation function cr e -=φto the hydrogen atom; choose the parameter c to minimize the variational integral, and calculate the percent error in the ground-state energy. Solution :1) The requirement of the variation function being a well-behaved function requires that c must be a positive number.2) check the normalization of the variation function.322*)(c d d Sin dr r ed cr πϕθθτφφ==⎰⎰⎰⎰- 3) The variation integral equals to )2(214])2[(2)1(21()121(ˆ32223*32*32*3**-=-∂∂+∂∂-=-∇-=-∇-==⎰⎰⎰⎰⎰⎰--c c c dr r e rr r e c d r c c d r c d d H w cr cr τφφπφπτφφπτφφτφφ4) The minimum of the variation integral is21101-=⇒=⇒=-=∂∂w c c c w5) The percent error in the ground state is 0%2. If the normalized variation function x l 2/13)/3(=φ for 0 ≤ x ≤ l is applied to the particle-in-a-one-dimensional-box problem, one finds that the variation integral equals zero, which is less than the true ground-state energy. What is wrong?Solution:The correct trail variation function must be subject to the same boundary condition of the given problem. For the particle in a 1D box problem, the correct wavefunction must equal to zero at x=0 and x=l. However, the trial variation function x l 2/13)/3(=φ does not fulfill these requirement. The variation integral basedon this incorrect variation function does not make any sense.3. Application of the variation function2cx e -=φ(where c is a variation parameter) to a problem with V = af (x), where a is a positive constant and f (x ) is a certain function of x , gives the variation integral as W = c ħ2/2m +15a /64c 3. Find the minimum value of W for this variation function.Solution: 23434123434141min 4141413272598.03)25(23)25(0)64152(ηηηηm a m a w m a c dc c a m c d c w ==⇒±=⇒=+=∂∂4. In 1971 a paper was published that applied the normalized variation functionN exp(-br 2a 02-cr /a 0) to the hydrogen atom and stated that minimization of the variation integral with respect to the parameters b and c yielded an energy 0.7% above the true ground-state energy for infinite nuclear mass. Without doing any calculations, state why this result must be wrong.Solution:From the evaluation of exercise 1, we know that the variation function exp(-cr) gives no error in the ground state of hydrogen atom. This function is a special case of the normalized variation function N exp(-br 2a 02-cr /a 0) when b equals to zero. Therefore, adopting the normalized variation function as a trial variation function should also have no error in the ground state energy for hydrogen atom.5. Prove that, for a system with anondegenerate ground state, 0*ˆE d H>⎰τφφ, if φ is any normalized, well-behaved function that is not equal to the true ground-state wavefunction. (E 0 is the lowest-energy eigenvalueof Hˆ) Solution:As the eigenfunctions of the Hermitian operator H form a complete set, any well-behaved function which is subject to the same boundary condition can be expanded as a linear combination of the eigenfunction of the Hermitian operator, namely,∑∞==0i i i c ψφ, where ψi s are eigenfunctions of Hermitian operator H, c i s are constant.The expectation value of φ with respect to the Hermitian operator is00201020201020020*00**00*0*0*ˆˆ)(ˆE c E E c E c c E c E c E c c E c cH c c d c H c d H i i i i i i i i i i i i i ij j j j i i i j j i i j j j i i i ==+>+======∑∑∑∑∑∑∑⎰∑∑⎰∑∑⎰∞=∞=∞=∞=∞=∞=∞=∞=∞=∞=∞=δψψτψψτφφChapter 09, 101. For the anharmonic oscillator with Hamiltonian 43222212ˆdx cx kx dx d m H +++-=η, evaluate E (1)for the first excited state, taking the unperturbed system as the harmonic oscillator. Solution:The wavefunction of the first excited state of the harmonic oscillator is241312)4(x xe απαψ-=Hence, the first order correct to energy of the first excited state is given by6213422134134324131'*1415)4()4()4)(()4(ˆ222απαπαπαπαψψαααd dx e x d dx x d e x xe x d x c xe dx H x x x ==•=•+•=---⎰⎰⎰⎰2. Consider the one-particle, one-dimensional system with potential-energyV = V 0 for l x l 4341<<, V = 0 for l x 410≤≤ andl x l ≤≤43and V = ∞ elsewhere, where V 0 = 22/ml η.Treat the system as a perturbed particle in a box. (a) Find the first-order energy correction for the general stationary state with quantum number n . (b) Find the first-order correction to the wave function of the stationary state with quantum number n .Solution:The wavefunction of a particle in 1D box is given by)(2)0(x ln Sin l n πψ= Take this as unperturbed wavefunction, and the perturbation H ’ is given by V .a) The first-order energy correction for ψn is])23[]2[(224]2[4[2)()(2)()(2ˆ00004341)0('*)0()1(πππππππππψψn Sin n Sin n V V n S n Sin l l V dx V x l n Sin x l n Sin l dx x ln VSin x l n Sin l dx H E l ln n -+=-+====⎰⎰⎰b) The first correction to the wavefunction is given by)0()0()0()0()0()1(2)0()0(222)0('(818mn m mn n m n mn n E E H n E E ml hn E ψψψψ∑∞≠-==-⇒=3. For an anharmonic oscillator with3222212ˆcx kx dx d m H ++-=η, take 'ˆHas cx 3. (a) Find E (1) for the state with quantum number v . (b) Find E (2) for the state with quantum number v . You will need the following integral:3,'2/13)0(3)0('2/)1[(3]8/)3)(2)(1[(||++++++>=<v v v v v v v v x αδαψψ3,'2/131,'2/3]8/)2)(1([)2/(3----++v v v v v v v v δαδαSolution: a) As the potential of the unperturbed is a even function, the eigenfunctions of the unperturbed system are either even or odd. The perturbation is an odd function with respect to x. Hence, the first order energy correction is zero.b) The second order energy correction is given by)51212(8]38)2)(1(838)1(338)3)(2)(1([()2(3)21(38)3)(2)(1((''2323333332)0()0(1,2/31,2/33,32)0()0(2)0(3)0()0()0()0()0()0()0()2(++-=--++-++-+++=-+++++++=-=-=∑∑∑∞≠-++∞≠∞≠n n hvc hvn n n hv n hv n hv n n n c E E n n n n n n n c E E cx E E H H E n m mn n n n n n n n m mn m n n m m n m n nm n αααααδαδαδαψψψψψψ4. Calculate the angle that the spin vector S makes with the z axis for an electron with spin function α.Solution:For an electron, both S and S z equal to one half. The magnitude of S isοηη74.54]31[23)1(===+=ArcCos S S S θ 5. (a) Show that 12ˆP and 23ˆP do not commutewith each other. (b) Show that 12ˆPand 23ˆP commute when they are applied to antisymmetric functions.Solution:a) Set the wavefunction to be)1()3()2()2()1()3(321321φφφφφφ≠b) When the function is antisymmetric, we haveψψψψψψψ2312121223231223ˆˆ)(ˆˆˆ)(ˆˆˆP P P P P P P P =-=-==-=-=6. Which of the following functions are (a) symmetric? (b) antisymmetric?(1) )2()1()2()1(ααg f ; (2) )]2()1()2()1()[2()1(αββα-f f ;(3) )3()2()1()3()2()1(βββf f f ; (4) )(21r r a e --;(5) )]1()2()2()1()][2()1()2()1([βαβα--f g g f ; (6) )(21221r r a e r +-. Solution:(2) is antisymmetric(3), (5) and (6) are symmetricChapter 11, 131. How many electrons can be put in each of the following: (a) a shell with principal quantum number n ; (b) a subshell with quantum numbers n and l ; (c) an orbital; (d) a spin-orbital?Solution:a) 2n 2, b) 2*(2l+1), c) 2, d) 12. Give the possible values of the total-angular-momentum quantum number J that result from the addition of angular momentum with quantum numbers (a) 3/2 and 4; (b) 2, 3, and 1/2Solution:Coupling between two angular momentums with quantum number j 1 and j 2 gives the possible quantum number J of the total angular momentum as:2121j j J j j +<<-a) The possible values are 11/2, 9/2, 7/2, 5/2b) The possible values are:11/2, 9/2, 9/2, 7/2, 7/2, 5/2, 5/2, 3/2, 3/2, 1/23. Find the terms that arise from each of the following electron configurations: (a) 1s22s22p63s23p5g; (b) 1s22s22p3p3d(c) 1s22s22p24dSolution:As fully-filled sub-shells do not contribution the total orbital and spin angular momentum, we can ignore the electrons in these sub-shells while considering the atomic terms. Hence, a) The atomic terms can be:3H, 1H, 3G, 1G, 3F, 1Fb) The atomic terms can be:4G, 2G, 4F, 2F, 4D, 2D, 4P, 2P, 4S, 2S4F 2F, 4D, 2D, 4P, 2P4D, 2D, 4P, 2P, 4S, 2Sc) The atomic terms can be:2G, 2F, 2D, 2P, 2S4F, 2F, 4D, 2D, 4P, 2P。
量子化学习题及参考答案

量子化学习题集及答案1、(1)求一维势箱中运动粒子的波函数。
(2)当势箱长度为10nm 时,度求粒子在以下区域出现的概率。
4.95<X<5.053l <X<23l 解:222ˆ2d Hm dx =-由schrodinger 方程可得:2221221222121221222222220(cos sin )222sin0220sin 000222sin08axd mEdxC bx C bxmEr i mEmEC x C x x l mEmEC C C mEmEmEC l C l l n n h E ml ψψψψψπ+=+=∴=+=+==+===其通解为=e (1)又或时,=0即: 得得 解得:将其2*22222000222sin sin()sin()sin ()12l l l n C xln n n dx C x C x dx C x dx l l lC C l n x lπψπππψψπψ====∴=⇒=∴=⎰⎰⎰代入方程(1)则有:将其正交归一: (2)5.05 5.0524.95 4.95 4.955.05 5.054.95 4.954.95 5.051021cos()222)sin()sin ()212122(cos())[(5.05 4.95)(sin( 5.05)sin( 4.95))]2x l nmn x n n n l x x dx x dx dxl l l l l l n l n n dx x dx l l l n l lππππρππππ<<=-===-=---⎰⎰⎰⎰⎰即:=得: 0.0121X 333l l ρρ=<<=同理: 时,2、电子在在L=1.00nm 长的势箱中运动,计算:(1)n=2与n=1之间,n=6与n=5之间的能量差。
解:22212222222182181812222222182181881.001,2213811*********,6651181108110810n h E ml l nm n n h h h E m m m n n h h h E m m m ------====∴=-===∴=-=3、一维势箱(势箱长为0.30nm )中的电子发射一个小频率为5.05×1015S —1,试求其跃迁始态与终态的量子数。
量子化学习题集

(2) x, x2, 3x21; (3) sinx, cosx;
(4) sinx, cosx, tanx;
(5)
sin2x, cos2x, 1;
(6) sinx, cosx, eix (7) sin2x, cos2y, 1
2.2 三维势箱中一粒子的波函数是下列那些算符的本征函数?
ˆ x (2)p ˆx (1) p
2ˆ2 ˆ2ˆ2ˆ2 ˆ xS ˆ y, S ˆy ˆ xS (2) 化简下面算符:S S z, S xS yS z
3.7 计算下列积分: ˆ z|0,0; (1)0,0|M ˆ +M ˆ |2,0; (4) 2,0|M 3.8 计算下列积分: ˆ z|py; (1) px|M ˆ +|py; (2) px|M ˆ y|px; (3) pz|M ˆ x|py (4)pz|M ˆ x|px (5) pz|M ˆ +|2,0; (2)2,1|M ˆ +|2,0 ˆ M (5) 2,0|M
x e
0
n qx
dx
n! q n 1
n 1, q 0 b0 b 0, n 1, 2,3... a 0, n 0,1, 2, ...
e
0 0
bx 2
dx
1 2 b
x
t
2 n bx 2
e
dx
(2n)! 2 n 1 2 n 1 2 n! b
判断下列函数那些是偶函数,那些是奇函数?
(1) sinx (2) cosx (3) tanx
(4) ex
(5) 12
(6)22x
2.6 对谐振子 v=1 的态,求粒子最可能的位置 2.7 对氢原子的基态,求(1) r 的平均值;(2) r 的最可几值;(3)求 2p 态的r 2.8 证明对于定态,T+V=E 2.9 计算氢原子基态的T,V 2.10 已知,力学量 A 的不确定度为A, A
量子化学习题及答案

量子化学习题及答案1.1998及2013年度诺贝尔化学奖分别授予了量子化学以及分子模拟领域的杰出贡献者,谈谈你的了解及认识。
答:1998年诺贝尔化学奖得主:瓦尔特·科恩和约翰·波普尔。
1964-1965年瓦尔特·科恩提出:一个量子力学体系的能量仅由其电子密度所决定,这个量比薛定谔方程中复杂的波函数更容易处理得多。
他同时还提供一种方法来建立方程,从其解可以得到体系的电子密度和能量,这种方法称为密度泛函理论,已经在化学中得到广泛应用,因为方法简单,可以应用于较大的分子。
沃尔特·库恩的密度泛函理论对化学作出了巨大的贡献。
约翰·波普尔发展了化学中的计算方法,这些方法是基于对薛定谔方程中的波函数作不同的描述。
他创建了一个理论模型化学,其中用一系列越来越精确的近似值,系统地促进量子化学方程的正确解析,从而可以控制计算的精度,这些技术是通过高斯计算机程序向研究人员提供的。
今天这个程序在所有化学领域中都用来作量子化学的计算。
2013年诺贝尔化学奖得主:马丁·卡普拉斯、迈克尔·莱维特、阿里耶·瓦谢勒。
他们为复杂化学系统创立了多尺度模型。
为研发了解和预测化学过程的强有力的计算机程序奠定了基础。
对于今天的化学家来说,计算机就像试管一样重要。
模拟过程是如此的真实以至于传统实验的结果也能被计算机预测出来。
多尺度复杂化学系统模型的出现无疑翻开了化学史的“新篇章”。
化学反应发生的速度堪比光速。
刹那间,电子就从一个原子核跳到另一个原子核,以前,对化学反应的每个步骤进行追踪几乎是不可能完成的任务。
而在由这三位科学家研发出的多尺度模型的辅助下,化学家们让计算机做“做帮手”来揭示化学过程。
20世纪70年代,这三位科学家设计出这种多尺度模型,让传统的化学实验走上了信息化的快车道。
2.谈谈你对量子化学中两种流派(VBT,MOT)的认识。
答:1926年,奥地利物理学家薛定谔(Schrodinger)建立了描述电子运动规律的波动方程。
量子化学复习题

Chapter1Chapter3/4求矩阵的本征值及其近似值例2.在F 表象中Q 算符为 ,求Q 的本征值和正交归一的本征函数。
F*ψF⎦⎤⎢⎣⎡-=33i i Q解:由 得即又C1,C2均不为0,则有 解得入1=4,入2=2当入1=4时,解得此时当入2=2时,解得 此时例3. 一个正交基构成了如下的哈密顿算符矩阵⎥⎥⎥⎦⎤⎢⎢⎢⎣⎡+⎥⎥⎥⎦⎤⎢⎢⎢⎣⎡-=c c c H 000000200030001 c 为常数1) 求H 2) 3) 比较(1)和(2解:(1)因为 023120030=----=---λλλλλc cc c c)3)(1[(---λλ212c +±(2)修正到二级近似<+=||''W E E E 21211c E -= 22213c E += 23-=c E (3))211(21222c c +±=+±2213c +=和)211(2c - 比较结果即可知。
Chapter4 会写slater 行列式λψψ=Q 211=C i C 212=⎥⎥⎥⎥⎦⎤⎢⎢⎢⎢⎣⎡=ψ221)(i x 211=C 22iC -=⎥⎥⎤⎢⎢⎡=ψ21)(x ⎥⎦⎤⎢⎣⎡⎥⎦⎤⎢⎣⎡=⎥⎦⎤⎢⎣⎡=⎥⎦⎤⎢⎣⎡⎥⎦⎤⎢⎣⎡-2100212133C C C C C C i i λλλ033=---λλi iC H λ=ˆHe 基态原子的组态1S 2,其波函数为会算简单的行列式(二阶He 、三阶Li ) He 1S 2Li 1S 22S 1 和什么是变分原理?答:给定一个体系的哈密顿算符 ,如果 是任意一个合格条件下的函数,则有 E 0 (E 0为基态能量, 未归一化)E 0 (E 0为基态能量, 归一化) Chapter5())2(1)1(1)2(1)1(121ββααψs s s s =Hφ≥**=⎰⎰τφφτφφd d H Eφ≥*=⎰τφφd H Eφ())2(1)1(1)2(1)1(12121s s s s s s ϕϕϕϕψ=)3(1)2(2)1(2)3(1)2(1)1(1)3(1)2(1)1(161)321(αααβββαααψs s s s s s s s s =)3(1)2(2)1(2)3(1)2(1)1(1)3(1)2(1)1(161)321(ββββββαααψs s s s s s s s s =1.3、C 2h 群顺式丁二烯为例:基态:2221ψψ到第一激发态:131221ψψψ跃迁是否会产生紫外光谱?(分子平面为YZ 面)解:根据HMO 方法,得到其分子轨道432113717.06015.06015.03717.0φφφφψ+++= b 1 432126015.03717.03717.06015.0φφφφψ--+= a 2 432136015.03717.03717.06015.0φφφφψ+--= b 1432143717.06015.06015.03717.0φφφφψ-+-= a 2基态:2221ψψ 所属的不可约表示为:12211A a a b b =⊗⊗⊗ 第一激发态:131221ψψψ 所属的不可约表示为:21211B b a b b =⊗⊗⊗跃迁矩阵元的不可约表示*xx x dx μψμψ<>=⎰基态第一激发态 1122A B B A ∈⊗⊗= *yy y dy μψμψ<>=⎰基态第一激发态 1221A B B A ∈⊗⊗= *zz z dz μψμψ<>=⎰基态第一激发态 1122A A B B ∈⊗⊗=所以,上述跃迁会产生紫外光谱。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
单电子近似是认为每个电子都是在诸原子核的静电场及其它电子的有效平均场中“独立 地”运动着。于是,在该电子的势能函数中,其它电子的坐标都在对电子排斥能求平均的过 程中被除掉了,唯独只剩下各项电子自己的坐标作为变量,这样在哈密顿算符中,既考虑了
e2 的项,又同时在形式上把它变成和其他电子的相对位置无关。于是体系中每个电子都在
ei
i H eff i i i
可得出以下结论和公式:
ei e10 e i Δ12
e i S 12 Δ12
e
i
e
0 2
0
ei e10 ei e20 eiS12 Δ12 2 0 ①
因为C2H4的分子轨道情况是兼并的,所以 e10 e20
由①可得到
e10 ei
2
12 e1S12
可容纳电子对数
1
3
5
它们与sp、sp2、sp3杂化轨道的主要不同在于杂化轨道比未杂化的原子轨道的成键能力
强。原子之所以要发生杂化,是因为生成的杂化轨道比未杂化的原子轨道有更强的成键能力
和更合理的空间取向,从而有利于原子间在合理的位置形成稳定的化学键,生成更稳定的分
子。
9. 何为分子轨道?何为原子轨道?
i
Z1
1
a rai 2 i r ji ij
在单电子近似下可得到 Hˆ H eff (ri ,i ,i ) i
即: Hˆ E H eff i eii 2 (i为单电子波函数)
由平均值公式
ei
i H eff i i i
可得出以下结论和公式:
ei e10 ei Δ12
(1)分子轨道是通过相应的原子轨道线性组合而成的轨道。有几个原子轨道相结合就形
成几个分子轨道。在组合产生的分子轨道中,能量低于原子轨道的称为成键轨道,高于原子
轨道则为反键轨道。无对应的原子轨道直接生成的称为非成键轨道。
(2)原子轨道是单电子薛定谔方程的合理解 ψ(x,y,z)。若用球坐标来描述这组解,
原理。 (2)在量子化学计算中,广泛采用的是线性变分函数,它是满足体系边界条件的 m 个
线性无关的函数1 ,2 ,……m 的线性组合: c11 c2 2c3 3 cm m 这里常用实 函数作为变分函数。采用线性变分函数的变分法通常称线性变分法。
15. 使用分子轨道理论求乙烯分子、2-丁烯分子的成键分子轨道能级 e1 和分子轨道波函数
Py
图(1) ③原子 A 的 Pz 原子轨道与原子 B 的 dyz 原子轨道形成 型分子轨道(如图 2)
图(2) 3.原子 A 和原子 B 在 y 轴上,指出 A 原子的 px, py, pz 原子轨道与 B 原子的 dxy, dyz, dz2 原 子轨道间分别可形成什么分子轨道? ①原子 A 的 Px 原子轨道与原子 B 的 dxy 原子轨道形成π型分子轨道(如图 3)。
Na+的薛定谔方程
Hˆ
h2 8 2m
10 i 1
i2
10 i 1
11e 2 4 0ri
10 i 1
i j
e2 4 0rij
Hˆ E
F-的薛定谔方程:
Hˆ
h2 8 2m
10
i 2
i1
10 i1
9e2 40ri
10 i1
i j
e2 40rij
Hˆ E
5.写出任意一个分子的能量算符。写出H2、H2O和C6H6的薛定谔方程算符表达式。
1 2
10
i 2
i1
10 i1
1 rH1i
10 i1
1 rH2i
10 i1
8 rOi
10 i1
i j
1 rij
8 Ra1
8 Ra2
1 Ra3
Hˆ E
C6H6定核近似下的薛定谔方程表达式为:
Hˆ
1 2
42
i 2
i1
6
a1
42 i1
6 rai
6
b1
42 i1
1 rbi
42
i1
i j
1 rij
基础量子化学习题 1.为什么微观粒子的运动状态要使用量子力学来描述,而不能用经典力学来描述? 答:以牛顿三大定律为中心内容的经典力学适用于宏观物体的机械运动,当经典力学的应用 范围推广到高速运动和小线度范围时,实验表明经典力学遇到了不可克服的困难。对微观体 系的研究导致了量子力学的诞生。因为许多微观粒子都具有波粒二象性。而在观察之前,我 们得不到一个粒子的确切位置,它是一道弥散的波。可以通过波函数来表示它们在每一区域 出现的概率。所以,描述描述微观粒子的运动状态要用量子力学。 2.原子 A 和原子 B 在 y 轴上,指出 A 原子的 Px, Py, Pz 原子轨道与 B 原子的 dxy, dyz, dz2 原子轨道间分别可形成什么分子轨道? ①原子 A 的 Px 原子轨道与原子 B 的任意原子轨道无法形成分子轨道 ②原子 A 的 Py 原子轨道与原子 B 的 dyz 原子轨道形成π分子轨道(如图 1)
H2定核近似下能量算符表达式为:
Hˆ
1 2
12 22
1 ra1
1 rb1
1 ra 2
1 rb 2
1 r12
1 R
H2定核近似下的薛定谔方程表达式为:
E
1 2
12 22
1 ra1
11 rb1 ra2
1 rb 2
1 r12
1 R
H2O定核近似下的薛定谔方程表达式为:
Hˆ
②原子 A 的 Py 原子轨道与原子 B 的任意原子轨道都无法形成分子轨道。 ③原子 A 的 Pz 原子轨道与原子 B 的 dyz 原子轨道形成 型分子轨道(如图 4)。
4.写出任意一个原子的能量算符。写出 Na+和 F-的薛定谔方程算符表达式。
H 原子的能量算符: Hˆ
h2 8 2
2
e2 4 0 r
2
其键为分子轨道能量 E ,因为轨道只有两个电子。
又因为: 1 为成键轨道,成键轨道能量低,使分子稳定,所以两电子都排布在 1 轨道上。
E 2e1 2e10 即键键能为 2e1 2e10
其在原子核区和键区域的电子布局:
16. 使用分子轨道理论求甲醛分子、一氧化碳分子的成键分子轨道能级 e1 和分子轨道波函
数 ,并求键键能以及在原子核区域和键区域的电子布居。据此讨论共价键的本质。 (1)甲醛分子式为CH2O,C原子与O原子可以形成一个 键和一个键。其中的 键与键是 相互分离的并无干扰作用。
由分子轨道的波函数 i c1i 1 c2i 2 i 1,2
由能量算符 Hˆ
i
1 2
2 i
由分子轨道的波函数 i c1i 1 c2i 2 i 1,2
有能量算得: Hˆ
i
1 2
2 i
i
a
Z rai
1 2
i
ji
1 rij
在电子近似下可得到: Hˆ
H eff ( ri , i , i )
i
即: Hˆ E Heffi eii2(i为单电子波函)
由平均值公式
1 ,并求键键能以及在原子核区域和键区域的电子布居。据此讨论共价键的本质。
(1)乙烯 乙烯的分子式是C2H4,每个碳原子提供 4 个价电子,每个氢原子提供 1 个价电子,总共 12 个价电子。乙烯中所以原子都处在同一个平面上,因此所有的 键都在分子平面上。而两 个C原子p轨道则垂直于分子平面,它们形成键,在分子平面上的上下两边。由于 键是轴 对称的,键是面对称的,对称性不同故相应的分子轨道是属于不平均表示的基函数,相互之 间没有作用。故 键对键无作用。我们只讨论键即可。
ei S12 Δ12 ei e20
(2)共价键的形成是原子轨道或分子轨道的叠加,组成新的分子轨道,而不是电子云的 叠加。原子轨道有正有负,按波的规律叠加,有的加强有的减弱,形成成键分子轨道或反键 分子轨道。
(3)从能量角度看,聚集在核间运动的电子,同时受到两个核正电荷的吸引,降低体系 能量,有利于电子在核间聚集。
(4)从简并和非简并轨道上看,在简并和非简并轨道下,两轨道两电子相互作用比两轨 道四电子相互作用更稳定,且相互作用的能量随着电子云的重叠的增大而升高,从非简并轨 道作用下,重要的相互作用与相互作用轨道的能量差成反比,能量高的作用弱。 11.何为简并轨道?何为非简并轨道?
即x, y, z RrY 这里 Rr是与径向分布有关的函数,称为径向分布函数,用图形来
描述就是原子轨道的径向分布函数;Y 是与角度分布有关的函数,用图形来描述就是角
度分布函数。 10. 谈谈你对共价键本质的理解。
(1)当原子相互接近时,它们的原子轨道相互同号叠加,组合成成键分子轨道,当电子 进入成键轨道,体系能量降低,形成稳定的分子,此时原子间形成共价键。从电子在分子中 的分布情况,可了解共价键的成因,电子在分子中的分布,可由分子中空间各点概率密度数 值的大小表示。分子中电子的分布和两个原子的电子分布的简单加和不同,电子云分布的差 值图反映了这一结果,电子云分布的差值图是将按空间各点逐点的减去处在 A 核位置的和处 在 B 核位置后,给出差值线图。
6 i1
36 Rai
6 i1
