2018年AMC8考题和答案解析
2018年美国数学竞赛 AMC 试题

2018 AIME I ProblemsProblem 1Let be the number of ordered pairs ofintegers with and such that thepolynomial can be factored into the product of two (not necessarily distinct) linear factors with integer coefficients. Find the remainder when is divided by .Problem 2The number can be written in base as , can be written inbase as , and can be written in base as , where . Find the base- representation of .Problem 3Kathy has red cards and green cards. She shuffles the cards and laysout of the cards in a row in a random order. She will be happy if and only if all the red cards laid out are adjacent and all the green cards laid out are adjacent. For example, card orders RRGGG, GGGGR, or RRRRR will make Kathy happy,but RRRGR will not. The probability that Kathy will be happy is ,where and are relatively prime positive integers. Find . Problem 4In and . Point lies strictlybetween and on and point lies strictly between and on sothat . Then can be expressed in the form ,where and are relatively prime positive integers. Find .Problem 5For each ordered pair of real numbers satisfyingthere is a real number such thatFind the product of all possible values of .Problem 6Let be the number of complex numbers with the propertiesthat and is a real number. Find the remainder when is divided by .Problem 7A right hexagonal prism has height . The bases are regular hexagons with side length . Any of the vertices determine a triangle. Find the number of these triangles that are isosceles (including equilateral triangles).Problem 8Let be an equiangular hexagon suchthat , and . Denote the diameter of the largest circle that fits inside the hexagon. Find .Problem 9Find the number of four-element subsets of with the propertythat two distinct elements of a subset have a sum of , and two distinct elements of a subset have a sum of . Forexample, and are two such subsets.Problem 10The wheel shown below consists of two circles and five spokes, with a label at each point where a spoke meets a circle. A bug walks along the wheel, starting at point . At every step of the process, the bug walks from one labeled point to an adjacent labeled point. Along the inner circle the bug only walks in a counterclockwise direction, and along the outer circle the bug only walks in a clockwise direction. For example, the bug could travel along thepath , which has steps. Let be the number of paths with steps that begin and end at point . Find the remainder when is divided by .Problem 11Find the least positive integer such that when is written in base , its two right-most digits in base are .Problem 12For every subset of , let be the sum of the elements of , with defined to be . If is chosen at random among allsubsets of , the probability that is divisible by is , where and are relatively prime positive integers. Find .Problem 13Let have side lengths , , and .Point lies in the interior of , and points and are the incentersof and , respectively. Find the minimum possible areaof as varies along .Problem 14Let be a heptagon. A frog starts jumping at vertex . From any vertex of the heptagon except , the frog may jump to either of the two adjacentvertices. When it reaches vertex , the frog stops and stays there. Find the number of distinct sequences of jumps of no more than jumps that end at .Problem 15David found four sticks of different lengths that can be used to form three non-congruent convex cyclic quadrilaterals, , which can each be inscribed in a circle with radius . Let denote the measure of the acute angle made by the diagonals of quadrilateral , and define and similarly. Supposethat , , and . All three quadrilaterals have thesame area , which can be written in the form , where and are relatively prime positive integers. Find .2018 AMC 8 ProblemsProblem 1An amusement park has a collection of scale models, with ratio , of buildings and other sights from around the country. The height of the United States Capitol is 289 feet. What is the height in feet of its replica to the nearest whole number?Problem 2What is the value of the productProblem 3Students Arn, Bob, Cyd, Dan, Eve, and Fon are arranged in that order in a circle. They start counting: Arn first, then Bob, and so forth. When the number contains a 7 as a digit (such as 47) or is a multiple of 7 that person leaves the circle and the counting continues. Who is the last one present in the circle?Problem 4The twelve-sided figure shown has been drawn on graph paper. What is the area of the figure in ?Problem 5What is the valueof ?Problem 6On a trip to the beach, Anh traveled 50 miles on the highway and 10 miles on a coastal access road. He drove three times as fast on the highway as on the coastal road. If Anh spent 30 minutes driving on the coastal road, how many minutes did his entire trip take?Problem 7The -digit number is divisible by . What is the remainder when this number is divided by ?Problem 8Mr. Garcia asked the members of his health class how many days last week they exercised for at least 30 minutes. The results are summarized in the following bar graph, where the heights of the bars represent the number of students.What was the mean number of days of exercise last week, rounded to the nearest hundredth, reported by the students in Mr. Garcia's class?Problem 9Tyler is tiling the floor of his 12 foot by 16 foot living room. He plans to place one-foot by one-foot square tiles to form a border along the edges of the room and to fill in the rest of the floor with two-foot by two-foot square tiles. How many tiles will he use?Problem 10The of a set of non-zero numbers is the reciprocal of the average of the reciprocals of the numbers. What is the harmonic mean of 1, 2, and 4?Problem 11Abby, Bridget, and four of their classmates will be seated in two rows of three for a group picture, as shown.If the seating positions are assigned randomly, what is the probability that Abby and Bridget are adjacent to each other in the same row or the same column?Problem 12The clock in Sri's car, which is not accurate, gains time at a constant rate. One day as he begins shopping he notes that his car clock and his watch (which is accurate) both say 12:00 noon. When he is done shopping, his watch says 12:30 and his car clock says 12:35. Later that day, Sri loses his watch. He looks at his car clock and it says 7:00. What is the actual time?Problem 13Laila took five math tests, each worth a maximum of 100 points. Laila's score on each test was an integer between 0 and 100, inclusive. Laila received the same score on the first four tests, and she received a higher score on the last test. Her average score on the five tests was 82. How many values are possible for Laila's score on the last test?Problem 14Let be the greatest five-digit number whose digits have a product of . What is the sum of the digits of ?Problem 15In the diagram below, a diameter of each of the two smaller circles is a radius of the larger circle. If the two smaller circles have a combined area of square unit, then what is the area of the shaded region, in square units?Problem 16Professor Chang has nine different language books lined up on a bookshelf: two Arabic, three German, and four Spanish. How many ways are there to arrange the nine books on the shelf keeping the Arabic books together and keeping the Spanish books together?Problem 17Bella begins to walk from her house toward her friend Ella's house. At the same time, Ella begins to ride her bicycle toward Bella's house. They each maintain a constant speed, and Ella rides 5 times as fast as Bella walks. The distancebetween their houses is miles, which is feet, and Bella covers feet with each step. How many steps will Bella take by the time she meets Ella?Problem 18How many positive factors does have?Problem 19In a sign pyramid a cell gets a "+" if the two cells below it have the same sign, and it gets a "-" if the two cells below it have different signs. The diagram below illustrates a sign pyramid with four levels. How many possible ways are there to fill the four cells in the bottom row to produce a "+" at the top of the pyramid?Problem 20In a point is on with and Point ison so that and point is on so that What is the ratio of the area of to the area ofProblem 21How many positive three-digit integers have a remainder of 2 when divided by 6, a remainder of 5 when divided by 9, and a remainder of 7 when divided by 11?Problem 22Point is the midpoint of side in square and meets diagonal at The area of quadrilateral is What is the areaofProblem 23From a regular octagon, a triangle is formed by connecting three randomly chosen vertices of the octagon. What is the probability that at least one of the sides of the triangle is also a side of the octagon?Problem 24In the cube with opposite vertices and and are the midpoints of edges and respectively. Let be the ratio of the area of the cross-section to the area of one of the faces of the cube. What isProblem 25How many perfect cubes lie between and , inclusive?2018 AMC 10A ProblemsProblem 1What is the value ofProblem 2Liliane has more soda than Jacqueline, and Alice has more soda than Jacqueline. What is the relationship between the amounts of soda that Liliane and Alice have?Liliane has more soda than Alice.Liliane has more soda than Alice.Liliane has more soda than Alice.Liliane has more soda than Alice.Liliane has more soda than Alice.Problem 3A unit of blood expires after seconds. Yasin donates a unit of blood at noon of January 1. On what day does his unit of blood expire?Problem 4How many ways can a student schedule 3 mathematics courses -- algebra, geometry, and number theory -- in a 6-period day if no two mathematics courses can be taken in consecutive periods? (What courses the student takes during the other 3 periods is of no concern here.)Problem 5Alice, Bob, and Charlie were on a hike and were wondering how far away the nearest town was. When Alice said, "We are at least 6 miles away," Bob replied, "We are at most 5 miles away." Charlie then remarked, "Actually the nearest town is at most 4 miles away." It turned out that none of the three statements were true. Let be the distance in miles to the nearest town. Which of the following intervals is the set of all possible values of ?Problem 6Sangho uploaded a video to a website where viewers can vote that they like or dislike a video. Each video begins with a score of 0, and the score increases by 1 for each like vote and decreases by 1 for each dislike vote. At one point Sangho saw that his video had a score of 90, and that of the votes cast on his video were like votes. How many votes had been cast on Sangho's video at that point?Problem 7For how many (not necessarily positive) integer values of is the value of an integer?Problem 8Joe has a collection of 23 coins, consisting of 5-cent coins, 10-cent coins, and 25-cent coins. He has 3 more 10-cent coins than 5-cent coins, and the total value of his collection is 320 cents. How many more 25-cent coins does Joe have than 5-cent coins?Problem 9All of the triangles in the diagram below are similar to iscoceles triangle , inwhich . Each of the 7 smallest triangles has area 1, and has area 40. What is the area of trapezoid ?Problem 10Suppose that real number satisfies. What is the valueof ?Problem 11When fair standard -sided die are thrown, the probability that the sum of the numbers on the top faces is can be written as, where is a positive integer. What is ?Problem 12How many ordered pairs of real numbers satisfy the following system ofequations?Problem 13A paper triangle with sides of lengths 3, 4, and 5 inches, as shown, is folded so that point falls on point . What is the length in inches of the crease?Problem 14What is the greatest integer less than or equal toProblem 15Two circles of radius 5 are externally tangent to each other and are internally tangent to a circle of radius 13 at points and , as shown in the diagram. The distance can be written in the form , where and are relatively prime positive integers. What is ?Problem 16Right triangle has leg lengths and . Including and , how many line segments with integer length can be drawn from vertex to a point on hypotenuse ?Problem 17Let be a set of 6 integers taken from with the property that if and are elements of with , then is not a multiple of . What is the least possible values of an element inProblem 18How many nonnegative integers can be written in theformwhere for ?Problem 19A number is randomly selected from the set , and a number is randomly selected from . What is the probabilitythat has a units digit of ?Problem 20A scanning code consists of a grid of squares, with some of its squares colored black and the rest colored white. There must be at least one square of each color in this grid of squares. A scanning code is called if its look does not change when the entire square is rotated by a multiple of counterclockwise around its center, nor when it is reflected across a line joining opposite corners or a line joining midpoints of opposite sides. What is the total number of possible symmetric scanning codes?Problem 21Which of the following describes the set of values of for which thecurves and in the real -plane intersect at exactly points?Problem 22Let and be positive integers suchthat , , ,and . Which of the following must be a divisor of ?Problem 23Farmer Pythagoras has a field in the shape of a right triangle. The right triangle's legs have lengths 3 and 4 units. In the corner where those sides meet at a right angle, he leaves a small unplantedsquare so that from the air it looks like the right angle symbol. The rest of the field is planted. The shortest distance from to the hypotenuse is 2 units. What fraction of the field is planted?Problem 24Triangle with and has area . Let be the midpointof , and let be the midpoint of . The angle bisectorof intersects and at and , respectively. What is the area of quadrilateral ?Problem 25For a positive integer and nonzero digits , , and , let be the -digit integer each of whose digits is equal to ; let be the -digit integer each of whose digits is equal to , and let be the -digit (not -digit) integer each of whose digits is equal to . What is the greatest possiblevalue of for which there are at least two values of such that ?2018 AMC 10B ProblemsProblem 1Kate bakes a 20-inch by 18-inch pan of cornbread. The cornbread is cut into pieces that measure 2 inches by 2 inches. How many pieces of cornbread does the pan contain?Problem 2Sam drove 96 miles in 90 minutes. His average speed during the first 30 minutes was 60 mph (miles per hour), and his average speed during the second 30 minutes was 65 mph. What was his average speed, in mph, during the last 30 minutes?Problem 3In the expression each blank is to be filled in with one of the digits or with each digit being used once. How many different values can be obtained?Problem 4A three-dimensional rectangular box with dimensions , , and has faces whose surface areas are 24, 24, 48, 48, 72, and 72 square units. What is ?Problem 5How many subsets of contain at least one prime number?Problem 6A box contains 5 chips, numbered 1, 2, 3, 4, and 5. Chips are drawn randomly one at a time without replacement until the sum of the values drawn exceeds 4. What is the probability that 3 draws are required?Problem 7In the figure below, congruent semicircles are drawn along a diameter of a large semicircle, with their diameters covering the diameter of the large semicircle with no overlap. Let be the combined area of the small semicircles and be the area of the region inside the large semicircle but outside the small semicircles. The ratio is 1:18. What is ?Problem 8Sara makes a staircase out of toothpicks as shown:This is a 3-step staircase and uses 18 toothpicks. How many steps would be in a staircase that used 180 toothpicks?Problem 9The faces of each of 7 standard dice are labeled with the integers from 1 to 6. Let be the probability that when all 7 dice are rolled, the sum of the numbers on the top faces is 10. What other sum occurs with the same probability ?Problem 10In the rectangular parallelepiped shown, , , and . Point is the midpoint of . What is the volume of the rectangular pyramid with base and apex ?Problem 11Which of the following expressions is never a prime number when is a prime number?Problem 12Line segment is a diameter of a circle with . Point , not equal to or , lies on the circle. As point moves around the circle, the centroid (center of mass) of traces out a closed curve missing two points. To the nearest positive integer, what is the area of the region bounded by this curve?Problem 13How many of the first numbers in the sequence are divisible by ?Problem 14A list of positive integers has a unique mode, which occurs exactly times. What is the least number of distinct values that can occur in the list?Problem 15A closed box with a square base is to be wrapped with a square sheet of wrapping paper. The box is centered on the wrapping paper with the vertices of the base lying on the midlines of the square sheet of paper, as shown in the figure on the left. The four corners of the wrapping paper are to be folded up over the sides and brought together to meet at the center of the top of the box, point in the figure on the right. The box has base length and height . What is the area of the sheet of wrapping paper?Problem 16Let be a strictly increasing sequence of positive integers suchthat What is the remainderwhen is divided by ?Problem 17In rectangle , and . Points and lie on ,points and lie on , points and lie on , and points and lie on so that and the convex octagon is equilateral. The length of a side of this octagon can be expressed in the form , where , , and are integers and is not divisible by the square of any prime. What is ?Problem 18Three young brother-sister pairs from different families need to take a trip in a van. These six children will occupy the second and third rows in the van, each of which has three seats. To avoid disruptions, siblings may not sit right next to each other in the same row, and no child may sit directly in front of his or her sibling. How many seating arrangements are possible for this trip?Problem 19Joey and Chloe and their daughter Zoe all have the same birthday. Joey is 1 year older than Chloe, and Zoe is exactly 1 year old today. Today is the first of the 9 birthdays on which Chloe's age will be an integral multiple of Zoe's age. What will be the sum of the two digits of Joey's age the next time his age is a multiple of Zoe's age?Problem 20A function is defined recursivelyby and for allintegers . What is ?Problem 21Mary chose an even -digit number . She wrote down all the divisors of in increasing order fromleft to right: . At some moment Mary wrote as a divisor of . What is the smallest possible value of the next divisor written to the right of ?Problem 22Real numbers and are chosen independently and uniformly at random from the interval . Which of the following numbers is closest to the probability that and are the side lengths of an obtuse triangle?Problem 23How many ordered pairs of positive integers satisfy theequation where denotes the greatest common divisor of and , and denotes their least common multiple?Problem 24Let be a regular hexagon with side length . Denote by , , and the midpoints of sides , , and , respectively. What is the area of the convex hexagon whose interior is the intersection of the interiors of and ?Problem 25Let denote the greatest integer less than or equal to . How many real numbers satisfy the equation ?2018 AMC 12A ProblemsProblem 1A large urn contains balls, of which are red and the rest are blue. How many of the blue balls must be removed so that the percentage of red balls in the urn will be ? (No red balls are to be removed.)Problem 2While exploring a cave, Carl comes across a collection of -pound rocks worth each, -poundrocks worth each, and -pound rocks worth each. There are at least of each size. He can carry at most pounds. What is the maximum value, in dollars, of the rocks he can carry out of the cave?Problem 3How many ways can a student schedule 3 mathematics courses -- algebra, geometry, and number theory -- in a 6-period day if no two mathematics courses can be taken in consecutive periods? (What courses the student takes during the other 3 periods is of no concern here.)Problem 4Alice, Bob, and Charlie were on a hike and were wondering how far away the nearest town was. When Alice said, "We are at least 6 miles away," Bob replied, "We are at most 5 miles away." Charlie then remarked, "Actually the nearest town is at most 4 miles away." It turned out that none of the three statements were true. Let be the distance in miles to the nearest town. Which of the following intervals is the set of all possible values of ?Problem 5What is the sum of all possible values of for which the polynomials andhave a root in common?Problem 6For positive integers and such that , both the mean and the median ofthe set are equal to . What is ?Problem 7For how many (not necessarily positive) integer values of is the value of an integer?Problem 8All of the triangles in the diagram below are similar to iscoceles triangle , in which. Each of the 7 smallest triangles has area 1, and has area 40. What is the area of trapezoid ?Problem 9Which of the following describes the largest subset of values of within the closed interval forwhich for every between and , inclusive?How many ordered pairs of real numbers satisfy the following system of equations?Problem 11A paper triangle with sides of lengths 3,4, and 5 inches, as shown, is folded so that point falls on point . What is the length in inches of the crease?Problem 12Let be a set of 6 integers taken from with the property that if and are elements of with , then is not a multiple of . What is the least possible value of an element inProblem 13How many nonnegative integers can be written in the formwherefor ?Problem 14The solutions to the equation , where is a positive real number other thanor , can be written as where and are relatively prime positive integers. What is ?A scanning code consists of a grid of squares, with some of its squares colored black and therest colored white. There must be at least one square of each color in this grid of squares. A scanning code is called if its look does not change when the entire square is rotated by a multiple of counterclockwise around its center, nor when it is reflected across a line joining opposite corners or a line joining midpoints of opposite sides. What is the total number of possible symmetric scanning codes?Problem 16Which of the following describes the set of values of for which the curves andin the real -plane intersect at exactly points?Problem 17Farmer Pythagoras has a field in the shape of a right triangle. The right triangle's legs have lengths 3 and 4 units. In the corner where those sides meet at a right angle, he leaves a small unplanted squareso that from the air it looks like the right angle symbol. The rest of the field is planted. The shortest distance from to the hypotenuse is 2 units. What fraction of the field is planted?Triangle with and has area . Let be the midpoint of, and let be the midpoint of . The angle bisector of intersects and atand , respectively. What is the area of quadrilateral ?Problem 19Let be the set of positive integers that have no prime factors other than , , or . The infinite sumof the reciprocals of the elements of can be expressed as , where and are relatively primepositive integers. What is ?Problem 20Triangle is an isosceles right triangle with . Let be the midpoint ofhypotenuse . Points and lie on sides and , respectively, so thatand is a cyclic quadrilateral. Given that triangle has area , the length canbe written as , where , , and are positive integers and is not divisible by the square of any prime. What is the value of ?Problem 21Which of the following polynomials has the greatest real root?Problem 22The solutions to the equations and whereform the vertices of a parallelogram in the complex plane. The area of thisparallelogram can be written in the form where and are positive integersand neither nor is divisible by the square of any prime number. What isProblem 23In and Points and lie on sidesand respectively, so that Let and be the midpoints of segmentsand respectively. What is the degree measure of the acute angle formed by linesandProblem 24Alice, Bob, and Carol play a game in which each of them chooses a real number between 0 and 1. The winner of the game is the one whose number is between the numbers chosen by the other two players. Alice announces that she will choose her number uniformly at random from all the numbers between 0 and 1, and Bob announces that he will choose his number uniformly at random from all thenumbers between and Armed with this information, what number should Carol choose to maximize her chance of winning?Problem 25For a positive integer and nonzero digits , , and , let be the -digit integer each of whosedigits is equal to ; let be the -digit integer each of whose digits is equal to , and let bethe -digit (not -digit) integer each of whose digits is equal to . What is the greatest possible value of for which there are at least two values of such that ?2018 AMC 12B ProblemsProblem 1Kate bakes 20-inch by 18-inch pan of cornbread. The cornbread is cut into pieces that measure 2 inches by 2 inches. How many pieces of cornbread does the pan contain?Problem 2Sam drove 96 miles in 90 minutes. His average speed during the first 30 minutes was 60 mph (miles per hour), and his average speed during the second 30 minutes was 65 mph. What was his average speed, in mph, during the last 30 minutes?Problem 3A line with slope 2 intersects a line with slope 6 at the point . What is the distance between the -intercepts of these two lines?Problem 4A circle has a chord of length , and the distance from the center of the circle to the chord is . What is the area of the circle?Problem 5How many subsets of contain at least one prime number?Suppose cans of soda can be purchased from a vending machine for quarters. Which of the following expressions describes the number of cans of soda that can be purchased for dollars, where 1 dollar is worth 4 quarters?Problem 7What is the value ofProblem 8Line segment is a diameter of a circle with . Point , not equal to or , lies on the circle. As point moves around the circle, the centroid (center of mass) of traces out a closed curve missing two points. To the nearest positive integer, what is the area of the region bounded by this curve?Problem 9What isProblem 10A list of positive integers has a unique mode, which occurs exactly times. What is the least number of distinct values that can occur in the list?Problem 11。
2018年全国高中数学联合竞赛试题及解答.(A卷)

{}{}{}{}∈⎢,3⎥,即OQ∈[1,3],6⨯6=36种,从而abc+def为奇数的概率为722018年全国高中数学联合竞赛一试(A卷)一、填空题:本大题共8个小题,每小题8分,共64分。
2018A1、设集合A=1,2,3, ,99,集合B=2x|x∈A,集合C=x|2x∈A,则集合B C 的元素个数为◆答案:24★解析:由条件知,B C=2,4,6, ,48,故B C的元素个数为24。
2018A2、设点P到平面α的距离为3,点Q在平面α上,使得直线PQ与平面α所成角不小于300且不大于600,则这样的点Q所构成的区域的面积为◆答案:8π★解析:设点P在平面α上的射影为O,由条件知tan∠OQP=OP⎡3⎤OQ⎣3⎦所以区域的面积为π⨯32-π⨯12=8π。
2018A3、将1,2,3,4,5,6随机排成一行,记为a,b,c,d,e,f,则abc+def是偶数的概率为◆答案:9 10★解析:先考虑abc+def为奇数时,abc,def一奇一偶,①若abc为奇数,则a,b,c为1,3,5的排列,进而d,e,f为2,4,6的排列,这样共有6⨯6=36种;②若abc为偶数,由对称性得,也有119=,故所求为1-=6!1010102018A4、在平面直角坐标系xOy中,椭圆C:x2y2+a2b2=1(a>b>0)的左右焦点分别是F,F,12椭圆C的弦ST与U V分别平行于x轴和y轴,且相交于点P,已知线段PU,PS,PV,PT的长分别为1,2,3,6,则∆PF F的面积为12★解析:由对称性,不妨设点 P x , y在第一象限,则 x = PT -PS 即 P 2,1 。
进 而 可 得 U2,2 , S 4,1 , 代 入 椭 圆 方 程 解 得 : a 2 = 20 , b 2 = 5 , 从 而 2 2[ ]◆答案: π - 2,8 - 2π ][ ] [ ][ ] 所以 π - 2 < x < 8 - 2π ,即不等式的解集为 π - 2,8 - 2π ] ⎩bx 2 - 2bx = 0◆答案: 15()2 = 2 ,y 0 =PV - PU2= 1( ) ( ) ( )S ∆PF 1F2=1 1F F ⨯ y = ⨯ 2 15 ⨯ 1 = 15 。
amc8试题

amc8试题AMC 8试题第一题1. 在一盘巨大的沙漏中,向上面的玻璃瓶中积分有10多克,此后倒转沙漏,沿相同的管道均匀地下降。
五分钟后,沙漏的底部以每分钟多少克的速度吐出沙子? (A) 2 (B) 4 (C) 5 (D) 10 (E) 12解答:这道题考察的是单位换算和平均速度的概念。
首先要注意题目中的单位换算,从“分钟”转换为“克”。
沙漏中积分的10克流失掉后,下降的总时间是五分钟,所以平均每分钟下降的克数就是10克除以五分钟,即2克/分钟。
因此,答案是 (A) 2。
第二题2. 将1到999的所以整数从小到大写下来,在所有数字中有多少个数字出现了至少一次? (A) 2701 (B) 3024 (C) 4000 (D) 5004 (E) 7381解答:这道题考察的是数字的计数和求和。
要找到所有1到999的整数中出现了至少一次的数字个数,我们可以分别计算每个位上的数字。
从个位开始,0出现了100次(0至999共100个数字),1出现了在个位上9次(10次)(1至991共100个数字,再加上100次),以此类推,9出现了100次(9至999共100个数字)。
因此,个位上的数字总共出现了10\*100次,即1000次。
同样的道理,我们可以得出十位上的数字总共出现了10\*100次,即1000次,百位上的数字总共出现了10\*100次,即1000次。
所以,所有1到999的整数中出现了至少一次的数字个数为1000+1000+1000,即3000个。
因此,答案是(B) 3024。
第三题3. 小明有6支不同颜色的铅笔和8张不同的宣纸。
一张宣纸必须用全相同颜色的铅笔画。
小明可以使用的方法数是多少? (A) 48 (B) 56 (C) 64 (D) 72 (E) 96解答:这道题考察的是排列组合。
小明有6种选择颜色的铅笔,对于每种颜色,有8张宣纸可供选择。
所以,总的方法数为6种颜色的选择乘以8张宣纸的选择,即6乘以8,得到答案为 (C) 48。
2018年AMC8考题和问题详解解析汇报
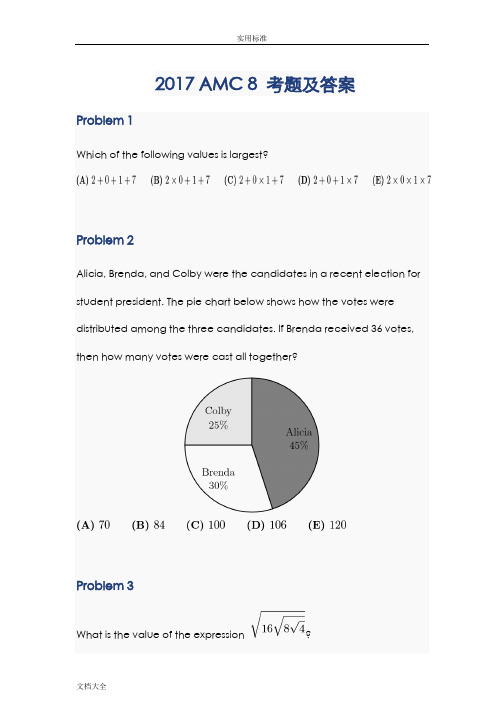
2017 AMC 8 考题及答案Problem 1Which of the following values is largest?Problem 2Alicia, Brenda, and Colby were the candidates in a recent election for student president. The pie chart below shows how the votes were distributed among the three candidates. If Brenda received 36 votes, then how many votes were cast all together?Problem 3What is the value of the expression ?Problem 4When 0.000315 is multiplied by 7,928,564 the product is closest to which of the following?Problem 5What is the value of the expression ?Problem 6If the degree measures of the angles of a triangle are in the ratio , what is the degree measure of the largest angle of the triangle?Problem 7Let be a 6-digit positive integer, such as 247247, whose first three digits are the same as its last three digits taken in the same order. Which of the following numbers must also be a factor of ?Problem 8Malcolm wants to visit Isabella after school today and knows the street where she lives but doesn't know her house number. She tells him, "My house number has two digits, and exactly three of the following four statements about it are true."(1) It is prime.(2) It is even.(3) It is divisible by 7.(4) One of its digits is 9.This information allows Malcolm to determine Isabella's house number. What is its units digit?Problem 9All of Marcy's marbles are blue, red, green, or yellow. One third of her marbles are blue, one fourth of them are red, and six of them are green. What is the smallest number of yellow marbles that Marcy could have?Problem 10A box contains five cards, numbered 1, 2, 3, 4, and 5. Three cards are selected randomly without replacement from the box. What is the probability that 4 is the largest value selected?Problem 11A square-shaped floor is covered with congruent square tiles. If the total number of tiles that lie on the two diagonals is 37, how many tiles cover the floor?Problem 12The smallest positive integer greater than 1 that leaves a remainder of 1 when divided by 4, 5, and 6 lies between which of the following pairs of numbers?Problem 13Peter, Emma, and Kyler played chess with each other. Peter won 4 games and lost 2 games. Emma won 3 games and lost 3 games. If Kyler lost 3 games, how many games did he win?Problem 14Chloe and Zoe are both students in Ms. Demeanor's math class. Last night they each solved half of the problems in their homework assignment alone and then solved the other half together. Chloe had correct answers to only of the problems she solved alone, but overall of her answers were correct. Zoe had correct answers to of the problems she solved alone. What was Zoe's overall percentage of correct answers?Problem 15In the arrangement of letters and numerals below, by how many different paths can one spell AMC8? Beginning at the A in the middle, a path allows only moves from one letter to an adjacent (above, below, left, or right, but not diagonal) letter. One example of such a path is traced in the picture.Problem 16In the figure below, choose point on so that and have equal perimeters. What is the area of ?Problem 17Starting with some gold coins and some empty treasure chests, I tried to put 9 gold coins in each treasure chest, but that left 2 treasure chests empty. So instead I put 6 gold coins in each treasure chest, but then I had 3 gold coins left over. How many gold coins did I have?Problem 18In the non-convex quadrilateral shown below, is a right angle, , , , and .What is the area of quadrilateral ?Problem 19For any positive integer , the notation denotes the product of the integers through . What is the largest integer for which is a factor of the sum ?Problem 20An integer between and , inclusive, is chosen at random. What is the probability that it is an odd integer whose digits are all distinct?Problem 21Suppose , , and are nonzero real numbers, and . What are the possible value(s) for ?Problem 22In the right triangle , , , and angle is a right angle. A semicircle is inscribed in the triangle as shown. What is the radius of the semicircle?Problem 23Each day for four days, Linda traveled for one hour at a speed that resulted in her traveling one mile in an integer number of minutes. Each day after the first, her speed decreased so that the number of minutes to travel one mile increased by 5 minutes over the preceding day. Each of the four days, her distance traveled was also an integer number of miles. What was the total number of miles for the four trips?Problem 24Mrs. Sanders has three grandchildren, who call her regularly. One calls her every three days, one calls her every four days, and one calls her every five days. All three called her on December 31, 2016. On how many days during the next year did she not receive a phone call from any of her grandchildren?Problem 25In the figure shown, and are line segments each of length 2, and . Arcs and are each one-sixth of a circle with radius 2. What is the area of the region shown?2017 AMC 8 Answer Key1. A2. E3. C4. D5. B6. D7. A8. D9. D10.C11.C12.D13.B14.C15.D16.D17.C18.B19.D20.B21.A22.D23.C24.D25.B。
全国中学生生物学联赛试题及答案及美国数学竞赛8年级(AMC8)真题(附答案)(电脑版)

全国中学生生物学联赛试题注意事项:1.所有试题使用2B铅笔在机读卡上作答;2.试题按学科分类,单选和多选题混排,多选题答案完全正确才可得分3.纸质试卷72题,电子试卷48题,共计l20题;4.答题时间120分钟。
一、细胞生物学、生物化学、微生物学16题1.癌细胞与正常细胞的不同之处在于A.癌细胞不能合成DNA B.癌细胞被锁定在细胞周期中的S期C.癌细胞能持续分裂尽管彼此紧密相接D.癌细胞始终处于细胞周期中的分裂期2.人的肌肉组织分为快缩纤维和慢缩纤维两种,快缩纤维负责剧烈运动如举重,短跑,易产生酸痛感觉;慢缩纤维负责慢跑,游泳等有氧运动。
以下关于慢缩纤维和快缩纤维的描述,哪个是正确的A.快缩纤维含有的线粒体多,有氧呼吸能产生大量乳酸和ATP供能B.慢缩纤维含有的线粒体多,有氧呼吸不产生乳酸,产生的ATP也少C.快缩纤维含有的线粒体少,主要依靠糖酵解产生ATP供能,因此产生大量乳酸D.慢缩纤维含有的线粒体多,主要依靠糖酵解产生ATP供能3.在光合作用中参与电子传递的载体是A.叶绿素B.磷酸烯醇式丙酮酸C.NADH D.NADPH4.肽链生物合成时,信号肽A.是线粒体的定位信号B.将新生肽链导入内质网C.控制蛋白质分子的最终构象D.处于肽链的C末端5.原核细胞的特征包括A.无核糖体B.无转录后修饰C.无有氧呼吸D.无染色体6.以下糖类中属于寡糖的有(多选2分)A.甘露糖B.麦芽糖C.半乳糖D.蔗糖E.糖原7.以下关于蛋白质变性后所产生现象的描述不正确的是:A.溶解度降低B.疏水侧链基团被包裹在分子内部C.形成沉淀D.失去结晶能力8.真菌中的半知菌是指。
A.没有光合作用B.菌丝没有横隔C.没有发现有性生殖阶段D.不能运动。
9.关于维生素A的生理功能,下面的描述中哪个是错误的?A.抑制胆碱酯酶活性B.构成视觉细胞感光物质的成分C.参与上皮组织细胞膜糖蛋白合成D.严重缺乏时导致夜盲症10.磷酸戊糖途径的发生部位在A.细胞质B.线粒体C.叶绿体D.细胞膜11.在C4植物循环中,CO2进入叶肉细胞被固定的最初产物是A.甘油酸-3-磷酸B.苹果酸C.草酰乙酸D.丙酮酸12.在等电聚焦电泳过程中,随着蛋白质样品的迁移,电流的变化为A.越变越大,当样品到达其等电点位置时,电流达到最大值B.越变越小,当样品到达其等电点位置时,电流达到最小值,接近于零C.基本不变,为一恒定值D.不确定13.利用酵母菌进行乙醇发酵时若通入氧气,会导致菌株对葡萄糖利用速度降低,甚至停止生成乙醇,这种现象称为A.呼吸抑制效应B.巴斯德效应C.分子氧效应D.葡萄糖效应14.巴斯德设计的曲颈瓶实验,可以(单选1分)A.证明酒是酵母菌发酵获得B.否定自然发生学说的论点C.说明培养基灭菌后的作用D.验证某细菌是不能培养的15.营养缺陷型菌株是指(单选1分)A.不需要添加生长因子可在基础培养基上生长的菌株B.不需要添加生长因子可在丰富培养基上生长的菌株C.因突变需提供某种营养才能在基础培养基上生长的菌株D.因自发或诱发突变而导致的可抵抗环境不良因素的菌株16.以下哪类化合物属于微生物的次生代谢产物(多选2分)A.脂肪B.色素C.抗生素D.蛋白质E.毒素二、植物和动物的解剖、生理、组织和器官18题17.草履虫、水螅、乌贼、蟾蜍受到刺激后,均可从体内发出一些物质以攻击或防御敌害,在他们身体上,发出这些物质的结构是A.刺丝泡、刺细胞、墨囊、耳后腺B.刺丝泡、刺丝囊、外套腔、唾液腺C.表膜泡、刺丝囊、墨囊、唾液腺D.表膜泡、刺细胞、外套腔、耳后腺18.在动物卵裂时期,由于不同动物受精卵内卵黄多少及其在卵内分布的不同,卵裂方式也有很大差异,海胆、沙蚕、昆虫、乌贼的卵裂方式依次分别为(单选1分) A.完全均等卵裂(等裂)、表面卵裂、螺旋形卵裂、盘裂B.螺旋形卵裂、完全均等卵裂(等裂)、盘裂、表面卵裂C.螺旋形卵裂、完全均等卵裂(等裂)、表面卵裂、盘裂D.完全均等卵裂(等裂)、螺旋形卵裂、表面卵裂、盘裂19.不同动物类群具有独特的特征,现存棘皮动物、海绵动物、哺乳动物、鸟类所特有的特征依次为A.水管系、水沟系、下颌为单一齿骨、羽毛B.后口、水沟系、胎生、飞翔C.后口、骨针、胎生、羽毛D.水管系、骨针、下颌为单一齿骨、飞翔20.节肢动物类群很多,不同类群的排泄器官亦有差异,节肢动物门甲壳纲动物的排泄器官有(多选l分)A.基节腺B.触角腺C.颚腺D.马氏管21.家鸽的一侧体动脉弓退化,雌家鸽的一侧卵巢和输卵管也退化了,退化的这些器官是(单选1分)A.左体动脉弓和右侧的卵巢、输卵管B.左体动脉弓和左侧的卵巢、输卵管C.右体动脉弓和左侧的卵巢、输卵管D.右体动脉弓和右侧的卵巢、输卵管22.在海滨潮间带经常可以见到石鳖和沙蚕,以下不属于它们共同特征的是A.以裂体腔法形成真体腔B.后肾型排泄系统C.具有担轮幼虫期D.开管式循环系统23.以下哪项不是文昌鱼的特征A.具有脊索,背神经管,鳃裂B.有分节的肌肉,有哈氏窝C.有头,有心脏D.有特化的口器24.一家饭店涉嫌出售野生鸟类,检查人员在检查时发现了一种鸟类的足,三趾向前一趾向后,后趾与前面三趾在同一平面上,趾长,基部有蹼相连,这种鸟类是A.鹈形目B.鹳形目C.雁形目D.鹤形目25.以下哪组元素在植物体内参与氧化还原反应(单选2分)A.钼镍铜铁B.铁铜镁钼C.钙镁镍铜D.锰镍镁铜26.盐胁迫条件下,较耐盐的禾本科植物大麦可以通过将盐分局域于以下部位来缓解盐分对植物生长造成的危害(多选l分)A.根系B.幼叶C.叶鞘D.老叶27.关于植物的种子,下列描述正确的是(多选2分)A.种子由胚珠发育而来B.种子表面均有种孔、种脐和种脊的结构C.种子中的胚乳多来源于受精后的中央细胞,也有来自于雌配子体的细胞D.胚是休眠的幼小孢子体E.无胚乳种子在发育过程中没有胚乳形成28.有关被子植物花的叙述,下列哪一个是错误的(单选2分)A.花是适应于繁殖功能的变态短枝B.花托、花萼和花冠被认为是叶的变态C.雄蕊和雌蕊也被认为是叶的变态D.花托、花被、雄蕊和雌蕊均有茎的顶端分生组织产生29.玉米干旱缺水时叶片的内卷主要是失水造成的A.叶肉细胞B.叶表皮的毛状体C.位于上表皮的泡状(运动)细胞D.位于下表皮的泡状(运动)细胞30.有关C4植物,以下说法中正确的是(多选2分)A.叶解剖结构中可观察到“花环结构”B.光合作用CO2的初步固定和同化在不同细胞中进行C.光合作用CO2的初步固定和同化在同一细胞中进行D.在一定范围的强光、高温条件下光合效率高31.心肌细胞有效不应期的长短主要取决于A.静息电位水平B.0期去极化的速度C.阈电位水平D.平台期的长短32.血液中CO2分压升高使呼吸运动加强的最主要途径是(单选2分)A.直接刺激脑桥的呼吸相关神经元B.直接刺激延髓呼吸中枢的神经元C.刺激中枢化学感受器D.刺激颈动脉体和主动脉体感受器33.当去甲肾上腺素与β受体结合时,下列哪一种肌肉收缩或收缩加强(单选1分) A.心室肌B.子宫平滑肌C.小肠平滑肌D.血管平滑肌E.支气管平滑肌34.下列哪种因素可引起人尿量的明显增加的(多选2分)A.献血200ml后B.饮用清水1000ml后C.静脉注射神经垂体激素D.饮用生理盐水100ml后三、动物行为学、生态学15题35.如果一项研究,专注于了解不同生态因子对生物的影响,及生物对它们的耐受,那么这个研究属于哪一层次上的研究A.个体生态学B.种群生态学C.群落生态学D.生态系统生态学36.关于高等动物种群中性别比例,下面论述中错误的是A.大多数种群倾向于使出生性比趋近于l:1 B.老年组往往雌性多于雄性C.出生的时候,往往雄性多于雌性D.种群性比与世代长度直接相关37.社会性寄生是指A.寄生在动物社会中是普遍现象B.寄生只发生在特定社会等级的动物中C.社会性昆虫中发生的寄生行为D.强迫寄主动物为其提供食物或其他利益38.关于外来物种,以下论述错误的是A.所有的外来物种都是入侵种,都是有害的B.外来物种可以依靠风、鸟、昆虫等自然因素入侵C.有些外来物种是人类有意引入的D.入侵物种可能对生态系统造成长久的破坏39.适合度是指A.动物单一行为的适应性B.动物调整自己的行为以适合于生活在当时的环境C.动物的总体繁殖成功性D.最适合动物生活习性、满足营养需求的食物40.以下哪种情况不属于动物的行为节律A.候鸟随季节的迁徙B.哺乳动物晨昏活动习性C.细菌生长速度随营养物浓度起落而快慢变化D.招潮蟹的活动随潮汐变化而变化41.动物的生长和发育是需要一定温度的,下列哪个说法是正确的(单选2分) A.外界温度的高低直接决定了动物机体的体温,进而影响其生长发育B.当外界温度低于某一温度时,昆虫就停止生长发育,这一温度阈值称为发育起点温度C.动物的发育速度总是随环境温度的增高而加快的D.昆虫发育的有效积温是发育历期乘以发育期的平均温度,然后求和42.下列有关水生群落演替的说法中哪个是错误的A.水生群落的演替一般会依次经历裸底期、浮水植物期、沉水植物期、挺水植物期、湿生草本植物期等阶段B.在这一演替过程中池底逐渐变浅,最终向陆地变化C.挺水植物根系往往较发达,可以使水底迅速增高D.浮水植物的叶子漂浮在水面,影响到水下光照,不利于沉水植物生长43.关于固定行为型,下述论述正确的是(多选2分)A.固定行为型被特定的外部刺激所释放B.每一个物种都有物种特异的固定行为型C.固定行为型一旦释放就会持续到底D.固定行为型是一种先天行为44.在动物行为学研究中,严格定义行为类型是研究工作的基础。
2018澳洲AMC中学高级组中文试题答案

: (D)
2. (A) 15 km
250 km (B) 20 km
8 × 30 = 240
(C) 30 km 30 km
8 (D) 40 km
(E) 80 km : (C)
5 Senior paper — draft for traditional chinese translation
3. (A) 16
11. 小
11-20
4
80
(
)
(A) 14
(B) 20
(C) 25
1 1 30 = 14 + 1 + 14 + 1
14 1
14
28
14
14
2 10 × 3 = 30
n
3n − 2(n − 1) = n + 2 n + 2 = 30
3n n−1
28
(D) 28 30 1
2
(E) 30 : (D) : (D)
218 = 29 × 29 < 1000 × 1000 = 106 : (A)
2018 = 262 144 000 000 000 000 000 000
23/8/2018
5 Senior paper — draft for traditional chinese translation
87
19.
88
20. 1◦
(A) 30
中 (B) 40
小 (C) 45
5 (D) 50
(E) 60
1 小
n
n 2
小
θ>0
n
n+5
(n − 2) × 180◦
180(n − 2) ◦ n
AMC8(美国数学竞赛)历年真题、答案及中英文解析

AMC8(美国数学竞赛)历年真题、答案及中英文解析艾蕾特教育的AMC8 美国数学竞赛考试历年真题、答案及中英文解析:AMC8-2020年:真题 --- 答案---解析(英文解析+中文解析)AMC8 - 2019年:真题----答案----解析(英文解析+中文解析)AMC8 - 2018年:真题----答案----解析(英文解析+中文解析)AMC8 - 2017年:真题----答案----解析(英文解析+中文解析)AMC8 - 2016年:真题----答案----解析(英文解析+中文解析)AMC8 - 2015年:真题----答案----解析(英文解析+中文解析)AMC8 - 2014年:真题----答案----解析(英文解析+中文解析)AMC8 - 2013年:真题----答案----解析(英文解析+中文解析)AMC8 - 2012年:真题----答案----解析(英文解析+中文解析)析)AMC8 - 2010年:真题----答案----解析(英文解析+中文解析)AMC8 - 2009年:真题----答案----解析(英文解析+中文解析)AMC8 - 2008年:真题----答案----解析(英文解析+中文解析)AMC8 - 2007年:真题----答案----解析(英文解析+中文解析)AMC8 - 2006年:真题----答案----解析(英文解析+中文解析)AMC8 - 2005年:真题----答案----解析(英文解析+中文解析)AMC8 - 2004年:真题----答案----解析(英文解析+中文解析)AMC8 - 2003年:真题----答案----解析(英文解析+中文解析)AMC8 - 2002年:真题----答案----解析(英文解析+中文解析)AMC8 - 2001年:真题----答案----解析(英文解析+中文解析)AMC8 - 2000年:真题----答案----解析(英文解析+中文解析)析)AMC8 - 1998年:真题----答案----解析(英文解析+中文解析)AMC8 - 1997年:真题----答案----解析(英文解析+中文解析)AMC8 - 1996年:真题----答案----解析(英文解析+中文解析)AMC8 - 1995年:真题----答案----解析(英文解析+中文解析)AMC8 - 1994年:真题----答案----解析(英文解析+中文解析)AMC8 - 1993年:真题----答案----解析(英文解析+中文解析)AMC8 - 1992年:真题----答案----解析(英文解析+中文解析)AMC8 - 1991年:真题----答案----解析(英文解析+中文解析)AMC8 - 1990年:真题----答案----解析(英文解析+中文解析)AMC8 - 1989年:真题----答案----解析(英文解析+中文解析)AMC8 - 1988年:真题----答案----解析(英文解析+中文解析)析)AMC8 - 1986年:真题----答案----解析(英文解析+中文解析)AMC8 - 1985年:真题----答案----解析(英文解析+中文解析)◆AMC介绍◆AMC(American Mathematics Competitions) 由美国数学协会(MAA)组织的数学竞赛,分为 AMC8 、 AMC10、 AMC12 。
2018年世界少年奥林匹克数学竞赛八年级海选赛试题含答案

绝密★启用前世界少年奥林匹克数学竞赛(中国区)选拔赛地方海选赛选手须知:1、本卷共三部分,第一部分:填空题,共计50分;第二部分:计算题,共计12分;第三部分:解答题,共计58分。
2、答题前请将自己的姓名、学校、赛场、参赛证号码写在规定的位置。
3、比赛时不能使用计算工具。
4、比赛完毕时试卷和草稿纸将被收回。
八年级试题(A卷)(本试卷满分120分 ,考试时间90分钟 )一、填空题。
(每题5分,共计50分)1、36的平方根是 。
2、若方程mx+ny=6的两个解是⎩⎨⎧==11y x 及⎩⎨⎧-==12y x ,则m= ,n = 。
3、已知1=-b a ,=+=+b a b a ,2522。
4、已知x=y+z=2,则=+++xyz z y x 333223。
5、如果实数a ,b 满足条件,12|21|,12222a b a b a b a -=+++-=+则a+b= 。
6、某班级春游时48人到杭州西湖划船。
已知每只小船坐3个人,租金16元;每只大船坐5个人,租金24元,则这个班级租金至少花 元。
7、在△ABC 中,∠B=60°,∠C >∠A ,且222B A )C ()()(∠+∠=∠,则△ABC 的形状是 。
8、观察下列式子:181092+⨯=;198100992+⨯=;199810009992+⨯=,……,按规律写出=2999999 。
(填写具体数字)9、如图,韩梅梅从A 点出发,沿直线前进10米后向左转30°,再沿直线前进10米,又向左转30°,照这样子走下去,他第一次回到起点A 时走了 米。
10、如图直线L 与∠A 的两边相交于点B 、C ,则图中以A 、B 、C 为端点的射线有 条。
二、计算题。
(每题6分,共计12分)11、 1+3+5+7+9+…+2017+201912、 1+5+52+53+…+5100省 市 学校 姓名 赛场 参赛证号∕∕∕∕∕〇∕∕∕∕∕∕〇∕∕∕∕∕∕∕〇∕∕∕∕∕∕ 密 〇 封 〇 装 〇 订 〇 线 ∕∕∕∕∕∕〇∕∕∕∕∕∕〇∕∕∕∕∕∕〇∕∕∕∕∕∕〇∕∕∕∕∕∕密 封 线 内 不 要 答 题三、解答题。
2018年AMC8考题和问题详解解析汇报
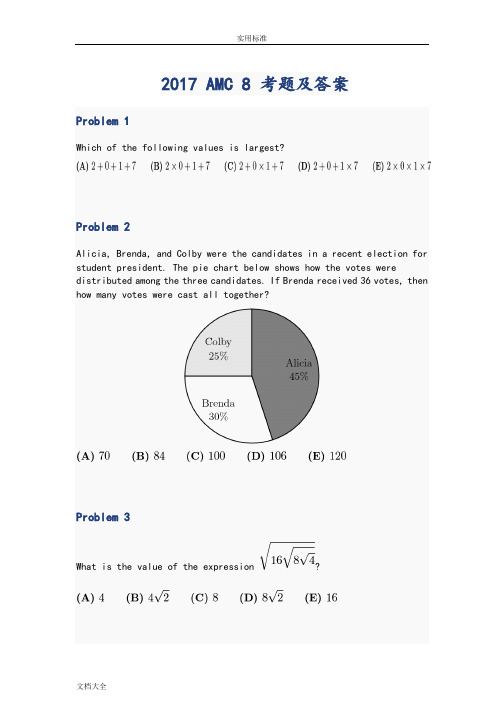
2017 AMC 8 考题及答案Problem 1Which of the following values is largest?Problem 2Alicia, Brenda, and Colby were the candidates in a recent election for student president. The pie chart below shows how the votes were distributed among the three candidates. If Brenda received 36 votes, then how many votes were cast all together?Problem 3What is the value of the expression ?Problem 4When 0.000315 is multiplied by 7,928,564 the product is closest to which of the following?Problem 5What is the value of the expression ?Problem 6If the degree measures of the angles of a triangle are in the ratio , what is the degree measure of the largest angle of the triangle?Problem 7Let be a 6-digit positive integer, such as 247247, whose first three digits are the same as its last three digits taken in the same order. Which of the following numbers must also be a factor of ?Problem 8Malcolm wants to visit Isabella after school today and knows the street where she lives but doesn't know her house number. She tells him, "My house number has two digits, and exactly three of the following four statements about it are true."(1) It is prime.(2) It is even.(3) It is divisible by 7.(4) One of its digits is 9.This information allows Malcolm to determine Isabella's house number. What is its units digit?Problem 9All of Marcy's marbles are blue, red, green, or yellow. One third of her marbles are blue, one fourth of them are red, and six of them are green. What is the smallest number of yellow marbles that Marcy could have?Problem 10A box contains five cards, numbered 1, 2, 3, 4, and 5. Three cards are selected randomly without replacement from the box. What is the probability that 4 is the largest value selected?Problem 11A square-shaped floor is covered with congruent square tiles. If the total number of tiles that lie on the two diagonals is 37, how many tiles cover the floor?Problem 12The smallest positive integer greater than 1 that leaves a remainder of 1 when divided by 4, 5, and 6 lies between which of the following pairs of numbers?Problem 13Peter, Emma, and Kyler played chess with each other. Peter won 4 games and lost 2 games. Emma won 3 games and lost 3 games. If Kyler lost 3 games, how many games did he win?Problem 14Chloe and Zoe are both students in Ms. Demeanor's math class. Last night they each solved half of the problems in their homework assignment alone and then solved the other half together. Chloe had correct answers to only of the problems she solved alone, but overall of her answers were correct. Zoe had correct answers to of the problems she solved alone. What was Zoe's overall percentage of correct answers?Problem 15In the arrangement of letters and numerals below, by how many different paths can one spell AMC8? Beginning at the A in the middle, a path allows only moves from one letter to an adjacent (above, below, left, or right, but not diagonal) letter. One example of such a path is traced in the picture.Problem 16In the figure below, choose point on so that and have equal perimeters. What is the area of ?Problem 17Starting with some gold coins and some empty treasure chests, I tried to put 9 gold coins in each treasure chest, but that left 2 treasure chests empty. So instead I put 6 gold coins in each treasure chest, but then I had 3 gold coins left over. How many gold coins did I have?Problem 18In the non-convex quadrilateral shown below, is a right angle, , , , and .What is the area of quadrilateral ?Problem 19For any positive integer , the notation denotes the product of the integers through . What is the largest integer for which is a factor of the sum ?Problem 20An integer between and , inclusive, is chosen at random. What is the probability that it is an odd integer whose digits are all distinct?Problem 21Suppose , , and are nonzero real numbers, and . What are the possible value(s) for ?Problem 22In the right triangle , , , and angle is a right angle. A semicircle is inscribed in the triangle as shown. What is the radius of the semicircle?Problem 23Each day for four days, Linda traveled for one hour at a speed that resulted in her traveling one mile in an integer number of minutes. Each day after the first, her speed decreased so that the number of minutes to travel one mile increased by 5 minutes over the preceding day. Each of the four days, her distance traveled was also an integer number of miles. What was the total number of miles for the four trips?Problem 24Mrs. Sanders has three grandchildren, who call her regularly. One calls her every three days, one calls her every four days, and one calls herevery five days. All three called her on December 31, 2016. On how many days during the next year did she not receive a phone call from any of her grandchildren?Problem 25In the figure shown, and are line segments each of length 2, and . Arcs and are each one-sixth of a circle with radius 2. What is the area of the region shown?2017 AMC 8 Answer Key1. A2. E3. C5. B6. D7. A8. D9. D10.C11.C12.D13.B14.C15.D16.D17.C18.B19.D20.B21.A22.D23.C24.D。
美国数学竞赛AMC8 -- 2018年真题解析(英文解析+中文解析)

美国数学竞赛AMC8 -- 2018年真题解析(英文解析+中文解析)Problem 1Answer: ASolution:You can just do 289/20 and round your answer to get 14.中文解析:比例是1:20 ,真实建筑是289feet,因此复制品的高度是: 289/20约等于14feet. 答案是A. Problem 2Answer: DSolution:By adding up the numbers in each of the 6 parentheses, wehave:(2/1)*(3/2)*(4/3)*(5/4)*(6/5)*(7/6) . Using telescoping, most of the terms cancel out diagonally. We are left with 7 .中文解析:=(2/1)*(3/2)*(4/3)*(5/4)*(6/5)*(7/6)=7/1=7. 答案是D.Problem 3Answer: DSolution:The five numbers which cause people to leave the circle are 7, 14, 17, 21,27. The most straightforward way to do this would be to draw out the circle with the people, and cross off people as you count. The last one standing is Dan.中文解析:依次离开圆圈的数字分别是7, 14, 17, 21, 27,... 直接把A, B, C, D, E 按圆形排列, 依次数, 划掉出局的, 最后剩下的是D. 答案是D.Problem 4Answer: CSolution:We count 3*3=9 unit squares in the middle, and 4 small triangles each with an area of 1. Thus, the answer is 9+4=13.中文解析:这个12边形围起来的小正方形共有9个,其面积是9*1=9. 小三角形有8个,其面积是8*0.5=4. 因此面积一共是9+4=13. 答案是C.Problem 5Answer: ESolution:We can rewrite the given expression as1+(3-2)+(5-4)+...+(2017-2016)+(2019-2018)=1+1+1+ (1)The number of 1s is the same as the number of terms in 1,3,5,7, ……2017, 2019. Thus the answer is 1010.中文解析:把上式按下面的规则用小括号括起来:=1+(3-2)+(5-4)+(7-6)+...+(2017-2016)+(2019-2018)=1+1+1+1+...+1+1.除了第一个1外,还有: 2018/2=1009个1. 因此和是1010. 答案是E.Problem 6Answer: CSolution:Since Anh spends half an hour to drive 10 miles on the coastal road, his speed is 10/0.5=20 mph. His speed on the highway then is 20*3=60 mph. He drives 50 miles, so he drives for 5/6 hours, which is equal to 50 minutes. The total amount of minutes spent on his trip is 30+50=80 minutes.中文解析:海滨道路上花费的时间是30分钟(0.5小时),路程是10英里,因此速度是: 10/0.5=20 . 则高速路的速度是3*20=60 mph. 高速路的距离是50mile, 则时间是50/60=5/6 小时=50分钟. 答案是A.Problem 7Answer: BSolution:We use the property that the digits of a number must sum to a multiple of 9 if it are divisible by 9. This means u=7. Since we are looking for the remainder when divided by 8, we can ignore the thousands. The remainder when 187 is divided by 8 is 3.中文解析:因为这个数能被9整除,因此 2+0+1+8+U 是9的倍数,因此u=7. 20187除以8的余数只需要看后三位187除以3的余数. 余数是3. 答案是B.Problem 8Answer: CSolution:The mean, or average number of days is the total number of days divided by the number of students. The total number of days is (1*1+2*3+3*2+4*6+5*8+6*4+7*2)=109. The total number of students is 1+3+2+6+8+3+2=25. Hence, 109/25=4.36.中文解析:有1个学生锻炼了1天, 3个学生锻炼了2天, 2个学生锻炼了3天, ....平均值是: (1+3*2+2*3+6*4+8*5+3*6+2*7)/(1+3+2+6+8+3+2)=109/25=4.36 答案是C. Problem 9Answer: BSolution:He will place (12*2)+(14*2)=52 tiles around the border. For the inner part of the room, we have 10*14=140 square feet. Each tile takes up 4 square feet, so he will use 140/4=35 tiles for the inner part of the room. Thus, the answer is 52+35=87.中文解析:1-foot 的正方形需要: 16*2+10*2=52块.中间剩下的面积是: 14*10=140. 2-foot的瓷砖的面积是2*2=4. 需要140/4=35块瓷砖.共需要: 52+35=87块瓷砖. 答案是B.Problem 10Answer: CSolution:The sum of the reciprocals is 1/1 + 1/2 +1/4=7/4. Their average is 7/12. Taking the reciprocal of this gives 12/7.中文解析:harmonic mean是先计算这组数的倒数的算数平均值,然后再求倒数. 1/1, 1/2, 1/4 的算数平均值是: 7/12. 其倒数是12/7. 因此harmonic mean是12/7. 答案是C.Problem 11Answer: CSolution:The total number of ways is C(6,2)=15, if we treat Abby and Bridget as a pair and distinguishable and forget the others. The total number of ways they are adjacent = 4 (for the rows) + 3 (for the columns) Therefore, P(Abby and Bridget sitting adjacent) is 7/15.中文解析:第一步安排A的座位, 第二步安排B的座位.A的座位可能位于4个角落之一,也可能位于两个中间位置之一. ,分两种情况.第一种, A的座位位于4个角落之一的可能性是4/6, 下一步安排B的座位, B和A相邻的可能性有: 2/5.第二种, A的座位唯一两个中间位置之一的可能性是2/6, 下一步安排B的座位, B和A相邻的可能性有: 3/5.综合起来: 4/6*2/5 + 2/6 * 3/5 =7/15. 答案是C.Problem 12Answer: BSolution:We see that every 35 minutes the clock passes, the watch passes 30 minutes. That means that the clock is 7/6 as fast the watch, so when the car clock passes 7 hours, the watch has passed 6 hours, meaning that the time would be 6:00.中文解析:当实际时间过了30分钟时(从12:00 到12: 30), 她的汽车表的时间过了35分钟. 其比值时30:35=6:7. 当汽车表显示7个小时时, 根据比例关系,实际时间应该是6点. 答案是B. Problem 13Answer: ASolution:Say Lalia gets a value of x on her first 4 tests, and a value of y on her last test. Thus, 4x+y=410.The value y has to be greater than 82, because otherwise she would receive the same score on her last test. Additionally, the greatest value for y is 98 (as y=100 would give x as a decimal), so therefore the greatest value y can be is 98. As a result, only 4 numbers work, 86, 90, 94, 98.中文解析:假设前4次考试的分数都是x, 最后一次考试的分数是y, 则4x+y=82*5. 即4x=410-y.其中x,y都是正整数, 82<y<=100. 并且410-y必须是4的倍数.y最大可以是98, 因此每次减4,y依次可以是98, 94, 90, 86. 共4种可能性. 答案是A. Problem 14Answer: DSolution:120=2*2*2*3*5. For the greatest five-digit number , 120=8*5*3*1*1. Thus, n is 85311. The sum of each digits is 8+5+3+1+1=18.中文解析:120=2*2*2*3*5. 为了这个5位数最大,首先让万位数上的数字最大, 120可以写成8*5*3*1*1. 因此这个5位数是85311. 其各位数字之和是: 8+5+3+1+1=18. 答案是D.Problem 15Answer: DSolution:Let the radius of the large circle be R. Then the radii of the smaller circles are R/2. The areas of the circles are directly proportional to the square of the radii, so the ratio of the area of the small circle to the large one is 1/4. This means the combined area of the 2 smaller circles is half of the larger circle, and therefore the shaded region is equal to the combined area of the 2 smaller circles, which is 1.中文解析:两个小圆面积合起来是1, 那么一个小圆的面积是0.5. 大圆的半径是小圆的半径的2倍,因此大圆的面积是小圆面积的4倍,大圆的面积是0.5*4=2. 阴影部分的面积是: 2-1=1. 答案是D.Problem 16Answer: CSolution:Since the Arabic books and Spanish books have to be kept together, we can treat them both as just one book. That means we're trying to find the number of ways you can arrange one Arabic book, one Spanish book, and three German books, which is just 5 factorial. Now we multiply this product by 2! because there are 2 factorial ways to arrange just the Arabic books, and 4! ways to arrange just the Spanish books. Multiplying all these together, we have 5!*2!*4!=5760.中文解析:用捆绑法,把2本Arabic 书看做一个整体, 4本Spanish书看做一个整体。
2018年全国高中数学联赛试题及答案详解(A卷)

2,
4,
6,,
48
,
故 B C 的元素个数为 24 . 2. 设点 P 到平面 的距离为 3 ,点 Q 在平面 上,使得直线 PQ 与 所成
角不小于 30 且不大于 60 ,则这样的点 Q 所构成的区域的面积为
.
答案:8 .
解:设点 P 在平面 上的射影为 O .由条件知,OP OQ
tan
OQP
3, 3求的区域面积为 32 12 8 .
3. 将1, 2, 3, 4, 5, 6 随机排成一行,记为 a, b, c, d , e, f ,则 abc + def 是偶数的
概率为
.
答案: 9 . 10
在[9,) 上严格递减,且 f (3) 0, f (9) 1,故结合图像可知
a (0, 3) , b (3, 9) , c (9, ) ,
并且 f (a) f (b) f (c) (0, 1) .
…………………4 分
由 f (a) f (b) 得 1 log3 a log3 b 1,
注意到 f ( 2) f () 1, f (8 2) f (2) f (2) 2 ,
所以 1 f (x) 2 f ( 2) f (x) f (8 2) ,
而1 2 8 2 2 ,故原不等式组成立当且仅当 x [ 2, 8 2] . 6. 设复数 z 满足 z 1,使得关于 x 的方程 zx2 2zx 2 0 有实根,则这样
证明: (1) 约定 S0 0 .由条件知,对任意正整数 n ,有
1
an
(2Sn
2018年全美数学竞赛amc8第13题

2018年全美数学竞赛amc8第13题
2018年全美数学竞赛AMC8第13题
在2018年全美数学竞赛AMC8中,第13题是一个比较有意思的
数学问题,它考察了考生对于几何图形的理解和计算能力。
这道题
目可以帮助考生提高对于几何图形的认识,同时也可以锻炼他们的
逻辑思维和解题能力。
题目描述如下:在一个正方形的对角线上,取一点P,连接PA,PB两条线段,使得PA:PB=1:2。
如果正方形的边长为6,那么线段
PA的长度是多少?
解题思路如下: 1. 首先,我们可以利用正方形的对角线的性质,即对角线的长度等于边长的平方根乘以2,来求得对角线的长度。
即对角线的长度为6*√2。
2. 然后,我们可以利用PA:PB=1:2
的比例关系,来求得线段PA的长度。
假设PA的长度为x,则PB的
长度为2x。
根据比例关系,我们可以得到x+2x=6*√2,即
3x=6*√2,解得x=2*√2。
因此,线段PA的长度为2*√2。
通过解答这道题目,考生可以加深对于几何图形的认识,同时也可以提高解题的能力。
在平时的学习中,考生可以多做类似的几何题目,加强对于几何知识的掌握,提高解题的能力。
总之,这道题目不仅可以帮助考生提高数学解题能力,也可以帮助他们加深对于几何知识的理解。
希望考生在备战数学竞赛的过程中,能够认真对待每一道题目,不断提升自己的数学水平。
2018专八真题解析

2018年专八试卷核对试卷一PAKl K THANSLATiO^ Trurthk/iv the “皿川曲 part u>f rhe ic.rf frtm (r hitfestr intf* Frf 剧汕 Write叭川 x/afs^n /MF A V.s fJ /: f( \HEET 1HHEE 觀沁丈学F5■耐码使我们的内心町以达剽这柑的:想:荐癖、敏聽相走感•生适丕姐童血龙華书■蛤技们從供广川以迖對 神比现实里是好的境界巴印幣驭血的朮⑷能比我0」规沁從中晖小芒--1,无比空『凤实中的天姜孟: 览实中從有完羌的爱惯.但在节甲仃氷山的(栄'j- tn 勺祝葵佇』唧(汐町殴与朱fflui • 会弥补找«M 实生活中所存件的不2018TEM8作文:追求完美好还是不好ps :作文为材料作文,两则材料,材料主题为“追求完美好还是不好”1. formal innovation2. rapport3. atte nding sect ion4. writing long papers5. high nu mbers6. being filmed7. comparable questio ns8. a n atural order9. figure out10. se nsitive11. repeat ing12.i ntegrate into13.lo gical or n atural14. edit ing[20 MINI15.fu ndame ntal eleme nts听力:1.The in itial letters of an easy-to-remember phrase2.[A] he's made up his mind to cha nge some of his passwords.3.in truders are patie nt eno ugh to compute.4.[D] The US takes up the leadi ng edge of tech no logy.5.[A] Why not to write dow n passwords on no tebooks6.[D] the developme nt of gen etic tests is out of people's expectati on.7.[C] misgivi ng.8.[A] improve self-discipline of the industry.9.[D] Alie nated.10.stre ngthe n its supervisi on with in limits.阅读:11.[C] they are lack ing in skills required by certa in jobs.12.[A] was a pion eer in the welfare state of Great Brita in.13.the frailties of huma n n ature.14.[D] appeara nee.15.[C] the pursuit of econo mic ben efit16.[A] was a famous tragic actor in his town.17.She was a girl of frail and weak body.18.the actresses were not available the n.19.[A] Domi nant.20.[D] was in agreeme nt with.21.[C] frow ns upo n22.[A] ben efited from the oppositi on party's bill.23.[D] Joh n McCain supports the bill due to his political sta ndpo int.24.deceitful阅读回答问题:25.Proposals should be directed to the journal office.26.The an alogy rests on the market economy 。
2018年专业英语八级真题及答案解析

2018年专业英语八级真题及答案解析(1~15/共15题)PART I LISTENING COMPREHENSIONSECTION A MINI-LECTUREIn this section you will hear a mini-lecture. You will hear the lecture ONCE ONLY. while listening, take notes on the important points. Your notes will not be marked, but yon will need them to complete a gap-filling task after the mini-lecture. when the lecture is over, yon will be given two minutes to check your notes, and another ten minutes to complete the gap-filling task on ANSWER SHEET ONE. Use the blank sheet for note-taking.Play00:0007:30VolumeLanguage and HumanityLanguage is powerful and it can help us do or get things as we wish.Language as a born traitLanguage has evolved only in__1__Comparison between chimpanzees and human beings: -Chimpanzees-use of tools: once seen as a sign of__2__-inability to__3__-tendency to__4__-Human beings-able to improve and build on__5__-able to__6__ideasLanguage and social learningProblem of social learning:__7__-Cause:-stealing others´ ideas by__8__-Solution:-__9__developed to share ideasResults-__10__made available to every individual-language as social technology to enhance__11__Language and the modern worldExistence of many different languages has led to-separation of cooperative groups-__12__-knowledge protection-slow flow of ideas and tendency toward__13__Globalization needs__14__.__15__hinder cooperation.Solution: one world with one language第1题第2题第3题第4题第5题第6题第7题第8题第9题第10题第11题第12题第13题第14题第15题下一题(16~20/共10题)SECTION BIn this section you will hear everything ONCE ONLY. Listen carefully and then answer the questions that follow. Mark the correct answer to each question on your ANSWER SHEET.Play00:0004:26Volume第16题A.Announcement of results.ck of a time schedule.C.Slowness in ballots counting.D.Direction of the electoral events.第17题A.Other voices within Afghanistan wanted so.B.The date had been set previously.C.All the ballots had been counted.D.The UN advised them to do so.第18题A.To calm the voters.B.To speed up the process.C.To stick to the election rules.D.To stop complaints from the loser.第19题A.Unacceptable.B.Unreasonable.C.Insensible.D.Ill-considered.第20题A.Supportive.B.Ambivalent.C.Opposed.D.Neutral.上一题下一题(21~25/共10题)SECTION BIn this section you will hear everything ONCE ONLY. Listen carefully and then answer the questions that follow. Mark the correct answer to each question on your ANSWER SHEET.Play00:0004:44Volume第21题A.Ensure the government includes all parties.B.Discuss who is going to be the winner.C.Supervise the counting of votes.D.Seek support from important sectors.第22题A.36% -24%.B.46%-34%.C.56%-44%.D.66%-54%.第23题A.Both candidates.B.Electoral institutions.C.The United Nations.D.Not specified.第24题A.It was unheard of.B.It was on a small scale.C.It was insignificant.D.It occurred elsewhere.第25题A.Problems in the electoral process.B.Formation of a new government.C.Premature announcement of results.D.Democracy in Afghanistan.上一题下一题(26~30/共14题)PART II READING COMPREHENSIONSECTION A MULTIPLE-CHOICE QUESTIONSIn this section there are several passages followed by fourteen multiple-choice questions. For each multiple-choice question, there are four suggested answers marked [A] , [B], [C] and [D]. Choose the one that you think is the best answer.In this section there are several passages followed by fourteen multiple-choice questions. For each multiple-choice question, there are four suggested answers marked [A] , [B], [C] and [D]. Choose the one that you think is the best answer.(1)"Britain´s best export," I was told by the head of the Department of Immigration in Canberra ,"is people. " Close on 100,000 people have applied for assisted passages in the first five months of that year, and half of these are eventually expected to migrate to Australia.(2)The Australians are delighted. They are keenly aware that without a strong flow of immigrants into the workforce the development of the Australian economy is unlikely to proceed at the ambitious pace currently envisaged. The new mineral discoveries promise a splendid future, and the injection of huge amounts of American and British capital should help to ensure that they are properly exploited, but with unemployment in Australia down to less than 1.3 percent, the government is understandably anxious to attract more skilled labor.(3)Australia is roughly the same size as the continental United States, but has only twelve million inhabitants. Migration has accounted for half the population increase in the last four years, and has contributed greatly to the country´s impressive economic development. Britain has always been the principal source—ninety per cent of Australians are of British descent, and Britain has provided one million migrants since the Second World War.(4)Australia has also given great attention to recruiting people elsewhere. Australians decided they had an excellent potential source of applicants among the so-called "guest workers" who have crossed their own frontiers to work in other parts of Europe. There were estimated to be more than four million of them, and a large number were offered subsidized passages and guaranteed jobs in Australia. Italy has for some years been the second biggest source of migrants, and the Australians have also managed to attract a large number of Greeks and Germans.(5)One drawback with them, so far as the Australians are concerned, is that integration tends to be more difficult. Unlike the British, continental migrants have to struggle with an unfamiliar language and new customs. Many naturally gravitate towards the Italian or Greek communities which have grown up in cities such as Sydney and Melbourne. These colonies have their own newspapers, their own shops, and their own clubs. Their inhabitants are not Australians, but Europeans.(6)The government´s avowed aim, however, is to maintain "a substantially homogeneous society into which newcomers, from whatever sources, will merge themselves". By and large, therefore, Australia still prefers British migrants, and tends to be rather less selective in their case than it is with others.(7)A far bigger cause of concern than the growth of national groups, however, is the increasing number of migrants who return to their countries of origin. One reason is that people nowadays tend to be more mobile, and that it is easier than in the past to save the return fare, but economic conditions also have something to do with it. A slower rate of growth invariably produces discontent—and if this coincides with greater prosperity in Europe, a lot of people tend to feel that perhaps they were wrong to come here after all.(8)Several surveys have been conducted recently into the reasons why people go home. One noted that "flies, dirt, and outside lavatories" were on the list of complaints from British immigrants, and added that many people also complained about " the crudity, bad manners, and unfriendliness of the Australians". Another survey gave climate conditions, homesickness, and " the stark appearance of the Australian countryside" as the main reasons for leaving.(9)Most British migrants miss council housing, the National Health scheme, and their relatives and former neighbors. Loneliness is a big factor, especially among housewives. The men soon make new friends at work, but wives tend to find it much harder to get used to a different way of life. Many are housebound because of inadequate public transport in most outlying suburbs, and regular correspondence with their old friends at home only serves to increase their discontent. One housewife was quoted recently as saying: " I even find I miss the people I used to hate at home. "(10)Rents are high, and there are long waiting lists for Housing Commission homes. Sickness can be an expensive business and the climate can be unexpectedly rough. The gap between Australian and British wage packets is no longer big, and people are generally expected to work harder here than they do at home. Professional men over forty often have difficulty in finding adecent job. Above all, perhaps, skilled immigrants often find a considerable reluctance to accept their qualifications.(11)According to the journal Australian Manufacturer, the attitude of many employers and fellow workers is anything but friendly. " We Australians," it stated in a recent issue, " are just too fond of painting the rosy picture of the big, warm-hearted Aussie. As a matter of fact, we are so busy blowing our own trumpets that we have not got time to be warm-hearted and considerate. Go down ´ heart-break alley´ among some of the migrants and find out just how expansive the Aussie is to his immigrants. "第26题The Australians want a strong flow of immigrants because______.A.immigrants speed up economic expansionB.unemployment is down to a low figureC.immigrants attract foreign capitalD.Australia is as large as the United States第27题Australia prefers immigrants from Britain because______.A.they are selected carefully before entryB.they are likely to form national groupsC.they easily merge into local communitiesD.they are fond of living in small towns第28题In explaining why some migrants return to Europe the author______.A.stresses their economic motivesB.emphasizes the variety of their motivesC.stresses loneliness and homesicknessD.emphasizes the difficulties of men over forty第29题Which of the following words is used literally, not metaphorically?A.flow(Para. 2).B.injection(Para. 2).C.gravitate(Para. 5).D.selective(Para. 6).第30题Para. 11 pictures the Australians as______.A.unsympatheticB.ungenerousC.undemonstrativeD.unreliable上一题下一题(31~35/共14题)PART II READING COMPREHENSIONSECTION A MULTIPLE-CHOICE QUESTIONSIn this section there are several passages followed by fourteen multiple-choice questions. For each multiple-choice question, there are four suggested answers marked [A] , [B], [C] and [D]. Choose the one that you think is the best answer.(1)Some of the advantages of bilingualism include better performance at tasks involving " executive function"(which involves the brain´s ability to plan and prioritize), better defense against dementia in old age and—the obvious—the ability to speak a second language. One purported advantage was not mentioned, though. Many multilinguals report different personalities, or even different worldviews, when they speak their different languages.(2)It´s an exciting notion, the idea that one´s very self could be broadened by the mastery of two or more languages. In obvious ways(exposure to new friends, literature and so forth)the self really is broadened. Yet it is different to claim—as many people do—to have a different personality when using a different language. A former Economist colleague, for example, reported being ruder in Hebrew than in English. So what is going on here?(3)Benjamin Lee Whorf, an American linguist who died in 1941, held that each language encodes a worldview that significantly influences its speakers. Often called " Whorfianism" , this idea has its sceptics, but there are still good reasons to believe language shapes thought.(4)This influence is not necessarily linked to the vocabulary or grammar of a second language. Significantly, most people are not symmetrically bilingual. Many have learned one language at home from parents, and another later in life, usually at school. So bilinguals usually have different strengths and weaknesses in their different languages—and they are not always best in their first language. For example, when tested in a foreign language, people are less likely to fall into a cognitive trap(answering a test question with an obvious-seeming but wrong answer)than when tested in their native language. In part this is because working in a second language slows down the thinking. No wonder people feel different when speaking them. And no wonder they feel looser, more spontaneous, perhaps more assertive or funnier or blunter, in the language they were reared in from childhood.(5)What of "crib" bilinguals, raised in two languages? Even they do not usually have perfectly symmetrical competence in their two languages. But even for a speaker whose two languages are very nearly the same in ability, there is another big reason that person will feel different in the two languages. This is because there is an important distinction between bilingualism and biculturalism.(6)Many bilinguals are not bicultural. But some are. And of those bicultural bilinguals, we should be little surprised that they feel different in their two languages. Experiments in psychology have shown the power of "priming"—small unnoticed factors that can affect behavior in big ways. Asking people to tell a happy story, for example, will put them in a better mood. The choice between two languages is a huge prime. Speaking Spanish rather than English, for a bilingual and bicultural Puerto Rican in New York, might conjure feelings of family and home. Switching to English might prime the same person to think of school and work.(7)So there are two very good reasons(asymmetrical ability, and priming)that make people feel different speaking their different languages. We are still left with a third kind of argument, though. An economist recently interviewed here at Prospero, Athanasia Chalari, said for example that:Greeks are very loud and they interrupt each other very often. The reason for that is the Greek grammar and syntax. When Greeks talk they begin their sentences with verbs and the form of the verb includes a lot of information so you already know what they are talking about after the first word and can interrupt more easily.(8)Is there something intrinsic to the Greek language that encourages Greeks to interrupt?People seem to enjoy telling tales about their languages´inherent properties, and how they influence their speakers. A group of French intellectual worthies once proposed, rather self-flatteringly, that French be the sole legal language of the EU, because of its supposedly unmatchable rigor and precision. Some Germans believe that frequently putting the verb at the end of a sentence makes the language especially logical. But language myths are not always self-flattering: many speakers think their languages are unusually illogical or difficult—witness the plethora of books along the lines of " Only in English do you park on a driveway and drive on a parkway: English must be the craziest language in the world!" We also see some unsurprising overlap with national stereotypes and self-stereotypes: French, rigorous: German, logical: English, playful. Of course.(9)In this case, Ms Chalari, a scholar, at least proposed a specific and plausible line of causation from grammar to personality: in Greek, the verb comes first, and it carries a lot of information, hence easy interrupting. The problem is that many unrelated languages all around the world put the verb at the beginning of sentences. Many languages all around the world are heavily inflected, encoding lots of information in verbs. It would be a striking finding if all of these unrelated languages had speakers more prone to interrupting each other. Welsh, for example, is also both verb-first and about as heavily inflected as Greek, but the Welsh are not known as pushy conversationalists.第31题According to the author, which of the following advantages of bilingualism is commonly accepted?A.Personality improvement.B.Better task performance.C.Change of worldviews.D.Avoidance of old-age disease.第32题According to the passage, that language influences thought may be related to______.A.the vocabulary of a second languageB.the grammar of a second languageC.the improved test performance in a second languageD.the slowdown of thinking in a second language第33题What is the author´s response to the question at the beginning of Para. 8?A.It´s just one of the popular tales of national stereotypes.B.Some properties inherent can make a language logical.C.German and French are good examples of Whorfianism.D.There is adequate evidence to support a positive answer.第34题Which of the following statements concerning Para. 9 is correct?A.Ms Chalari´s theory about the Greek language is well grounded.B.Speakers of many other languages are also prone to interrupting.C.Grammar is unnecessarily a condition for change in personality.D.Many unrelated languages don´t have the same features as Greek.第35题In discussing the issue, the author´s attitude is______.A.satiricalB.objectiveC.criticalD.ambivalent上一题下一题(36~39/共14题)PART II READING COMPREHENSIONSECTION A MULTIPLE-CHOICE QUESTIONSIn this section there are several passages followed by fourteen multiple-choice questions. For each multiple-choice question, there are four suggested answers marked [A] , [B], [C] and [D]. Choose the one that you think is the best answer.(1)Once across the river and into the wholesale district, she glanced about her for some likely door at which to apply. As she contemplated the wide windows and imposing signs, she became conscious of being gazed upon and understood for what she was—a wage-seeker. She had never done this thing before and lacked courage. To avoid conspicuity and a certain indefinable shame she felt at being caught spying about for some place where she might apply for a position, she quickened her steps and assumed an air of indifference supposedly common to one upon an errand. In this way she passed many manufacturing and wholesale houses without once glancing in. At last, after several blocks of walking, she felt that this would not do, and began to look about again, though without relaxing her pace. A little way on she saw a great door which for some reason attracted her attention. It was ornamented by a small brass sign, and seemed to be the entrance to a vast hive of six or seven floors. "Perhaps," she thought, "they may want someone" and crossed over to enter, screwing up her courage as she went. When she came within a score of feet of the desired goal, she observed a young gentleman in a grey clerk suit, fumbling his watch-chain and looking out. That he had anything to do with the concern she could not tell, but because he happened to be looking in her direction, her weakening heart misgave her and she hurried by, too overcome with shame to enter in. After several blocks of walking, in which the uproar of the streets and the novelty of the situation had time to wear away the effect of her first defeat, she again looked about. Over the way stood a great six-story structure labeled " Storm and King," which she viewed with rising hope. It was a wholesale dry goods concern and employed women. She could see them moving about now and then upon the upper floors. This place she decided to enter, no matter what. She crossed over and walked directly toward the entrance. As she did so two men came out and paused in the door. A telegraph messenger in blue dashed past her and up the few steps which graced the entrance and disappeared. Several pedestrians out of the hurrying throng which filled the sidewalks passed about her as she paused, hesitating. She looked helplessly around and then, seeing herself observed, retreated. It was too difficult a task. She could not go past them.(2)So severe a defeat told sadly upon her nerves. She could scarcely understand her weakness and yet she could not think of gazing inquiringly about upon the surrounding scene. Her feet carried her mechanically forward, every foot of her progress being a satisfactory portion of a flight which she gladly made. Block after block passed by. Upon street lamps at the various corners she read names such as Madison, Monroe, La Salle, Clark, Dearborn: and still she went, her feet beginning to tire upon the broad stone flagging. She was pleased in part that the streets were bright and clean. The morning sun shining down with steadily increasing warmth made theshady side of the streets pleasantly cool. She looked at the blue sky overhead with more realization of its charm than had ever come to her before.(3)Her cowardice began to trouble her in a way. She turned back along the street she had come, resolving to hunt up Storm and King and enter in. On the way she encountered a great wholesale shoe company, through the broad plate windows of which she saw an enclosed executive department, hidden by frosted glass. Without this enclosure, but just within the street entrance, sat a grey-haired gentleman at a small table, with a large open ledger of some kind before him. She walked by this institution several times hesitating, but finding herself unobserved she eventually gathered sufficient courage to falter past the screen door and stood humbly waiting.(4)"Well, young lady," observed the old gentleman, looking at her somewhat kindly—"what is it you wish?"(5)"I am, that is, do you—I mean, do you need any help?" she stammered.(6)"Not just at present," he answered smiling. "Not just at present. Come in sometime next week. Occasionally we need someone. "(7)She received the answer in silence and backed awkwardly out. The pleasant nature of her reception rather astonished her. She had expected that it would be more difficult, that something cold and harsh would be said—she knew not what. That she had not been put to shame and made to feel her unfortunate position seemed remarkable. She did not realize that it was just this which made her experience easy, but the result was the same. She felt greatly relieved.(8)Somewhat encouraged, she ventured into another large structure. It was a clothing company, and more people were in evidence.(9)An office boy approached her.(10)"Who is it you wish to see?" he asked.(11)"I want to see the manager," she returned.(12)He ran away and spoke to one of a group of three men who were conferring together. One broke off and came towards her.(13)"Well?" he said, coldly. The greeting drove all courage from her at once.(14)"Do you need any help?" she stammered.(15)"No," he replied abruptly and turned upon his heel.(16)She went foolishly out, the office boy deferentially swinging the door for her, and gladly sank into the obscuring crowd. It was a severe set-back to her recently pleased mental state.第36题She quickened her steps because she______.A.was afraid of being seen as a strangerB.was in a hurry to leave the districtC.wanted to look like someone working thereD.wanted to apply at more factories that day第37题Why didn´t she enter Storm and King the first time?A.She was too timid to enter the building.B.Two men stopped her at the entrance.C.Several pedestrians had found her strange.D.The messenger had closed the door behind him.第38题What does "every foot of her progress being a satisfactory portion of a flight which she gladly made" mean according to the context(Para. 2)?A.She thought she was making progress in job search.B.She was glad that she was looking for a job.C.She found her experience satisfactory.D.She just wanted to leave the place.第39题Why did she feel greatly relieved(Para. 7)?A.She eventually managed to enter the building.B.She was kindly received by the clerk.C.She had the courage to make an inquiry.D.She was promised a work position.上一题下一题(1/8)SECTION B SHORT-ANSWER QUESTIONSIn this section there are eight short-answer questions based on the passages in SECTION A. Answer each question in NO more than 10 words in the space provided.第40题What do "promise" and "should" in Para. 2 imply about the author´s vision of Australia´s economy? ______上一题下一题(2/8)SECTION B SHORT-ANSWER QUESTIONSIn this section there are eight short-answer questions based on the passages in SECTION A. Answer each question in NO more than 10 words in the space provided.第41题Explain the meaning of "the growth of national groups" according to the context(Para. 7). _______上一题下一题(3/8)SECTION B SHORT-ANSWER QUESTIONSIn this section there are eight short-answer questions based on the passages in SECTION A. Answer each question in NO more than 10 words in the space provided.第42题Explain the meaning of "The choice between two languages is a huge prime. " according to the context(Para. 6). _______上一题下一题(4/8)SECTION B SHORT-ANSWER QUESTIONSIn this section there are eight short-answer questions based on the passages in SECTION A. Answer each question in NO more than 10 words in the space provided.第43题What reasons does the author give to explain why people feel different when speaking different languages? _________上一题下一题(5/8)SECTION B SHORT-ANSWER QUESTIONSIn this section there are eight short-answer questions based on the passages in SECTION A.Answer each question in NO more than 10 words in the space provided.第44题What does the author focus on in the passage? _______上一题下一题(6/8)SECTION B SHORT-ANSWER QUESTIONSIn this section there are eight short-answer questions based on the passages in SECTION A. Answer each question in NO more than 10 words in the space provided.第45题Select and write down at least THREE words or phrases in Para. I describing the girl´s inner feelings while walking in the streets looking for a job. ______上一题下一题(7/8)SECTION B SHORT-ANSWER QUESTIONSIn this section there are eight short-answer questions based on the passages in SECTION A. Answer each question in NO more than 10 words in the space provided.第46题Explain the meaning of "So severe a defeat told sadly upon her nerves. " according to the context(Para. 2). _____上一题下一题(8/8)SECTION B SHORT-ANSWER QUESTIONSIn this section there are eight short-answer questions based on the passages in SECTION A. Answer each question in NO more than 10 words in the space provided.第47题In "It was a severe set-back to her recently pleased mental state. "(Para. 16), what does "her recently pleased mental state" refer to according to the context? _____上一题下一题(48~57/共10题)PART III LANGUAGE USAGEMass media is media that is intended for a large audience. Itmay take the form of broadcast media, as in case of television and__48__radio, or print media, as newspapers and magazines. __49__Usually, mass media aims to reach a very large market, such asthe entire population of a country. By contrast, local media covers amuch small population and area, focusing on regional news of__50__interest, specialty media is provided for particular demographic__51__groups. Some local media outlets that cover state or provincial newsmay raise to prominence thanks to their investigative journalism, and__52__to the clout that their particular regions have in the national politics.People often think of mass media as the news, it also includes__53__entertainment like television shows, books, and films. It may also beeducational in the nature, as in the instance of public broadcasting_54__stations that provide educational programs to a national audience.Political communications including propaganda are also frequentlydistributed through the media, as were public service announcements__55__and emergency alerts.When elitists may be tempted to sneer at mass media, referring__56__。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
2017 AMC 8 考题及答案Problem 1Which of the following values is largest?Problem 2Alicia, Brenda, and Colby were the candidates in a recent election for student president. The pie chart below shows how the votes were distributed among the three candidates. If Brenda received 36 votes, then how many votes were cast all together?Problem 3What is the value of the expression ?Problem 4When 0.000315 is multiplied by 7,928,564 the product is closest to whichof the following?Problem 5What is the value of the expression ?Problem 6If the degree measures of the angles of a triangle are in the ratio , what is the degree measure of the largest angle of thetriangle?Problem 7Let be a 6-digit positive integer, such as 247247, whose first three digits are the same as its last three digits taken in the same order. Which of the following numbers must also be a factor of ?Problem 8Malcolm wants to visit Isabella after school today and knows the street where she lives but doesn't know her house number. She tells him, "My house number has two digits, and exactly three of the following four statements about it are true."(1)It is prime.(2)It is even.(3)It is divisible by 7.(4)One of its digits is 9.This information allows Malcolm to determine Isabella's house number.What is its units digit?Problem 9All of Marcy's marbles are blue, red, green, or yellow. One third of her marbles are blue, one fourth of them are red, and six of them are green. What is the smallest number of yellow marbles that Marcy could have?Problem 10A box contains five cards, numbered 1, 2, 3, 4, and 5. Three cards are selected randomly without replacement from the box. What is theprobability that 4 is the largest value selected?Problem 11A square-shaped floor is covered with congruent square tiles. If the total number of tiles that lie on the two diagonals is 37, how many tiles cover the floor?Problem 12The smallest positive integer greater than 1 that leaves a remainder of 1 when divided by 4, 5, and 6 lies between which of the following pairs of numbers?Problem 13Peter, Emma, and Kyler played chess with each other. Peter won 4games and lost 2 games. Emma won 3 games and lost 3 games. If Kylerlost 3 games, how many games did he win?Problem 14Chloe and Zoe are both students in Ms. Demeanor's math class. Lastnight they each solved half of the problems in their homeworkassignment alone and then solved the other half together. Chloe had correct answers to only of the problems she solved alone, but overall of her answers were correct. Zoe had correct answers to of the problems she solved alone. What was Zoe's overall percentage of correct answers?WORD 资料 . 可编辑Problem 15In the arrangement of letters and numerals below, by how manydifferent paths can one spell AMC8? Beginning at the A in the middle, a path allows only moves from one letter to an adjacent (above, below,left, or right, but not diagonal) letter. One example of such a path is traced in the picture.Problem 16In the figure below, choose point on so that andhave equal perimeters. What is the area of ?WORD 资料 . 可编辑Problem 17Starting with some gold coins and some empty treasure chests, I tried toput 9 gold coins in each treasure chest, but that left 2 treasure chests empty. So instead I put 6 gold coins in each treasure chest, but then Ihad 3 gold coins left over. How many gold coins did I have?Problem 18In the non-convex quadrilateral shown below, is a right angle, , , , and .WORD 资料 . 可编辑What is the area of quadrilateral ?Problem 19For any positive integer , the notation denotes the product ofthe integers through . What is the largest integer for which is a factor of the sum ?Problem 20An integer between and , inclusive, is chosen at random.What is the probability that it is an odd integer whose digits are alldistinct?Problem 21Suppose , , and are nonzero real numbers, and . What are the possible value(s) for ?WORD 资料 . 可编辑Problem 22In the right triangle , , , and angle is a right angle. A semicircle is inscribed in the triangle as shown. What is theradius of the semicircle?Problem 23Each day for four days, Linda traveled for one hour at a speed thatresulted in her traveling one mile in an integer number of minutes. Eachday after the first, her speed decreased so that the number of minutesto travel one mile increased by 5 minutes over the preceding day. Eachof the four days, her distance traveled was also an integer number ofmiles. What was the total number of miles for the four trips?Problem 24WORD 资料 . 可编辑Mrs. Sanders has three grandchildren, who call her regularly. One callsher every three days, one calls her every four days, and one calls herevery five days. All three called her on December 31, 2016. On howmany days during the next year did she not receive a phone call fromany of her grandchildren?Problem 25In the figure shown, and are line segments each of length 2, and . Arcs and are each one-sixth of a circle with radius 2. What is the area of the region shown?WORD 资料 . 可编辑2017 AMC 8 Answer Key1. A2. E3. C4. D5. B6. D7. A8. D9. D10. C11. C12. D13. B14. C15. D16. D17. C18. B19. D专业技术 . 整理分享专业资料整理WORD 资料 . 可编辑20. B21. A22. D23. C24. D25. B专业技术 . 整理分享专业资料整理。
