2020年高考新题型填空题预测试题
2020届新高考英语新题型练习01 完形填空阅读理解七选五套餐练(1)(含答案)

2020届新高考英语新题型练习完形填空阅读理解七选五套餐练(1)完形填空(山东省临沂市2020年高三上学期期末考试)Three years ago,I participated in Model United Nations(MUN)held by the Education Department,which was a mock(模拟的)UN activity.During the two days,students are separated in different groups which 21 different countries to debate and try to solve problems.MUN has 22 awards:best delegates(代表),honorary mentions,and verbal mentions.Each time I participate in MUN,I made good 23 and undoubtedly got a few awards.But I often felt as if I’d 24 because I had never won the best delegate award.I 25 those who didn’t deserve to win the award but won it various times,and I was just filled with 26 at their success.But later I realized that it was also 27 not to get the award because I could actually 28 something,and that I shouldn’t 29 the best delegate award until I was the best delegate I could be.Failures are completely subjective—we can look at a result as a failure or a 30.Any failure can be regarded as a(n) 31 because you can always learn something from it and do 32 next time.This is supported by John Locke’s theory that we are born with blank views:knowledge and ability are learned from our 33.That’s true.If I make a mistake in the life practice,then I probably won’t 34 that next time.I believe this is 35 the best way to become better.21.A.strengthen B.condemn C.inspire D.represent 22.A.identical B.temporary C.various D.false 23.A.preparations B.predictions C.appointments D.explanations 24.A.accelerated B.failed C.exited D.succeeded 25.A.approached B.trained C.expected D.witnessed26.A.relief B.envy C.satisfaction D.delight 27.A.annoyed B.ashamed C.good D.terrible 28.A.desert B.1earn C.delete D.display29.A.win B.miss C.deliver D.value30.A.bond B.bet C.victory D.1oss31.A.aim B.benefit C.burden D.1imit32.A.funnier B.worse C.better D.slower33.A.decisions B.attitudes C.positions D.experiences 34.A.repeat B.admit C.accept D.notice 35.A.wrongly B.scarcely C.truly D.narrowly阅读理解(山东师大附中2017级第三次月考)AI was in the garden with Augie, my grandson, watching the bees. “How do they make honey?” Augie asked. “Actually, Augie, I don’t know,” I replied. “But, Grandma, you have your phone,” he said. For Augie, holding a smartphone almost means knowing everything.During my childhood I was crazy about books. Over time, reading hijacked my brain, as large areas once processing the real world adapted to processing the printed word. As far as I can tell, this early immersion (沉浸) didn’t prevent my development.Many parents worry that “screen time” will damage children’s development, but recent research suggests that most of the common fears about children and screens are unfounded. There is one exception: looking at screens before bed really disturbs sleep, in people of all ages. The American Academy of Pediatrics (AAP) used to recommend strict restrictions on screen exposure. Last year, the organization examined the relevant science more thoroughly and changed its recommendations. The new guidelines stress that what matters is what children watch and with whom.New tools have always led to panic guesses. The novel, the telephone, and the television were all declared to be the End of Civilization, particularly in the hands of the young. Part of the reason may be that adult brains require a lot of focus and effort to learn something new, while children’s brains are designed to master new environments naturally. New technologies always seem disturbing to the adults attempting to master them, while attractive to those children likeAugie.When Augie’s father got home, Augie rushed to meet him and said in excitement. “Daddy, Daddy, look,” he said, reaching for my phone. “Do you know how bees make honey? I’ll show you…”21. Which of the following can best replace the underlined word “hijacked” in Paragraph 2?A. occupied.B. damaged.C. improved.D. relaxed.22. What do the new guidelines of AAP focus on about “screen time”?A. The harm to children.B. The content and context.C. Children’s sleep.D. People’s fears.23. What might be the author’s attitude towards “screen time”?A. Opposed.B. Doubtful.C. Disappointed.D. Favorable.BToday companies have branches around the world. More than 11% of the US employers and employees work online either full-time or part-time, and that number is continuing growing. It is believed that it is a waste of time and money to fly around the world for face-to-face meetings. An effective solution to this problem is to use Web meetings.A large group of presentations, training classes and meetings are done online without losing the face-to-face experience. Web meetings are online meetings where an organizer invites attendees to listen to or watch an online presentation by presenters. Besides, Web meetings can be recorded for later use in presentations or training projects or downloaded for on-demand playback.Presenters can take real-time surveys to study how to hold a successful meeting. Some Web meeting software programs can monitor the users’ desktop behavior to see if they become distracted from the presentation and begin working on other documents. If so, the program can tell presenters when the listeners lose their attention, and how long the distraction lasts. In this way, the presenters will know which parts of their meetings need improving.Web meetings can work well because they’re hosted on a server (服务器). Images from the presenter’s desktop are taken, uploaded to a server and then downloaded by people who have accessto the server. Web meetings require a powerful server to deal with several images a second and “serve” them back to thousands of users at the same time.Companies have two choices when it comes to these servers. They can either buy a special Web meeting server to host their meetings on-site, or they can pay for a Web meeting service every time and let the off-site provider worry about hosting the meetings. The choice depends on how frequently the company holds Web meetings, the average number of people attending the meetings, and the quality of engineering and information technology.Web meetings are an excellent example of how technology is changing the way we do business. With all the technologies today, the traditional office might soon be a thing of the past.24.What is the disadvantage of the traditional meeting?A.It needs more people to organize it.B.It is expensive and time-consuming.C.It results in traffic accidents frequently.D.It fails to meet the demands of big companies.25.How do some Web meeting software programs help improve the meetings?A.By presenting successful documents.B.By recording the frequency of distraction.C.By tracking the listeners’ state of attention.D.By taking surveys about a successful meeting.26.What do you know about Web meetings according to the text?A.Web meetings are likely to be widely used.B.Web meetings help presenters stay focused.C.Web meetings determine the quality of engineering.D.Web meetings work well without the help of a server.CEvery year, thousands of teenagers participate in programs at their local art museums. But do any of them remember their time at museum events later in life? A new report suggests that the answer is yes – and finds that alumni (毕业生) of arts-based museum programs credit them with changing the course of their lives, even years after the fact.The Whitney Museum of American Art, the Walker Art Center and the Museum of Contemporary Art, Los Angeles recently asked researchers to conduct a study to find out how effective their long-standing teen art programs really are. They involved over 300 former participants of four programs for teens that have been in existence since the 1990s. Alumni, whose current ages range from 18 to 36, were invited to find out how they viewed their participation years after the fact.Among the alumni surveyed, 75 percent of alumni rated the teen program experience as the most favorable impact on their own lives, beating family, school and their neighborhoods. Nearly 55 percent thought t hat it was one of the most important experiences they’d ever had, regardless of age. And two-thirds said that they were often in situations where their experience in museums affected their actions or thoughts.It turns out that participating in art programs also helps keep teens enthusiastic about arts even after they reach adulthood: 96 percent of participants had visited an art museum within the last two years, and 68 percent had visited an art museum five or more times within the last two years. Thirty-two percent of program alumni work in the arts as adults.Though the study is the first of its kind to explore the impact of teen-specific art programs in museums, it reflects other research on the important benefits of engaging with the arts. A decade of surveys by the National Endowment for the Arts found that childhood experience with the arts is significantly associated with their income and educational attainment as adults. Other studies have linked arts education to everything from lower drop-out rates to improvement in critical thinking skills.27. What does the underlined phrase “the fact” in Paragraph 1 refer to?A. Changing the course of children’s life.B. Participating in childhood art programs.C. Organizing arts-based museum programs.D. Remembering the time at museum events.28. What does Paragraph 2 mainly tell?A. The result of the study.B. The process of the study.C. The approach to the study.D. The object and content of the study.29. What can be inferred of the study mentioned in the text?A. Passion for arts may remain long in kids’ whole life.B. No other studies exist concerning the benefits of arts.C. Age matters in how people view their art experiences.D. Most children taking part in art programs will work in arts.30. Which of the following can be the best title for the text?A. How is Art Connected to Our Life?B. Can Art Education Affect Our Income?C. What Should Art Museums do for Kids?D. Should Children Walk into Art Museums?七选五(山东师范大学附属中学2020届高三第三次月考)Words have the power to build us up or tear us down. It doesn’t matter if the words come from ourselves or someone else. The positive and negative effects are just as lasting.31 We’re usually too embarrassed to admit it, though. In fact, we really shouldn’t be because more and more experts believe talking to ourselves out loud is a healthy habit. This “self-talk” helps us motivate ourselves, remember things, solve problems, and calm ourselves down. Be aware, though, that as much as 77% of self-talk tends to be negative. 32Often, words come out of our mouths without us thinking about the effect they will have. But we should be aware that our words cause certain responses in others. For example, when returning an item to a store, we might use warm, friendly language during the exchange. And the clerk will probably respond in a similar manner. 33Words possess power because of their lasting effect. Many of us regret something we oncesaid. We remember unkind words said to us as well. Before speaking, we should always ask ourselves: 34 If what we want to say doesn’t pass this test, then it’s better left unsaid.Words possess power: both positive and negative. Those around us receive encouragement when we speak positively. We can offer hope, build self-esteem and motivate others to do their best. 35 Will we use our words to hurt or to heal? The choice is ours.A.Is it loving?B.How should I say it?C.We all talk to ourselves sometimes.D.Negative words destroy all those things.E.Generally people like positive and pleasant words.F.However, critical language may cause anger and defense.G.So we should only speak encouraging words to ourselves.参考答案完型填空21-25DCABD 26-30 BCBAC 31-35 BCDAC阅读理解21-25ABD BC 26-30 ABDAD七选五CGFAD。
2020新高考英语新题型练习05 完形填空阅读理解七选五套餐练(5)附答案

完形填空阅读理解七选五套餐练(5)完形填空(山东省潍坊市临朐2019-2020学年高三阶段性监测)I would like to send a huge shout out to Valerie, a manager at Home Depot. I, together with my son--- a boy with special needs, came in to 36 up a refrigerator box that she 37 for me. I was 38 to use it to build a police car for Halloween around my son’s wheelchair. When I explained the 39 to Valerie, she did not 40 to offer help. After getting the box, Valerie started to 41 down to cut the box for me. My son was happy to 42 her and they got along well with each other in doing the work.After we got the box loaded, Valerie helped us brainstorm and find the other 43 we would need. Then, as we neared the checkout, she had the entire order 44 . I tried to refuse but she just said that it would bless her 45 . My disabled son may not have understood anything 46 that Valerie was kind and patient with him, but I really appreciated this huge act of 47 . The biggest blessing for me was the way she 48 my son and the way he responded to her.Thanks to Valerie, Home Depot was also 49 their Saturday craft work-shops(手工艺作坊)into my son’s school. This way, our special kids could have 50 to a wonderful environment, where they could make their own crafts! Almost all kids in the class were 51 at the activities, which made them feel the value of themselves. It turned out to be 52 with all of them at last.I think one of the most 53 things that special-needs parents need to understand is that it’s OK to let other people in. Sometimes it’s hard to accept others’ 54 ; but when you refuse it, you 55 stand in the way of letting other people bless you and in turn that blesses them.36.A. build B. pick C. show D. cut37.A. kept B. bought C. made D. chose38.A. commanded B. planning C. studying D. instructed39.A. theory B. problems C. rules D. project40.A. hesitate B. pretend C. Agree D. Dare41.A. bend B. take C. turn D. put42.A. trust B. pity C. please D. join43.A. material B. methods C. ideas D. assistance44.A. changed B. shared C. paid D. arranged45.A. quickly B. privately C. quietly D. greatly46.A. along with B. next to C. instead of D. other than47.A. understanding B. kindness C. harmony D. politeness48.A. considered B. treated C. taught D. charged49.A. transforming B. adapting C. binging D. adding50.A. admission B. access C. attachment D. reaction51.A. embarrassed B. confused C. surprised D. excited52.A. demanding B. imaginary C. popular D. familiar53.A. difficult B. important C. pleasant D. meaningful54.A. respect B. guidance C. help D. invitation55.A. even B. clearly C. exactly D. actually阅读理解(湖北省十堰市2020年高三上学期元月调研考试)AStudent Scholarships5 Strong ScholarshipApplication Deadline: August 20thScholarship Description: The 5 Strong Scholarship Foundation is a team of experienced educators that have over 30 years of experience in helping minority nationality students get into college. We have teamed up to form a foundation that's going to be devoted to building groups of 5 college ready scholars and placing them on the campuses of Historically Black Colleges and Universities.Contact: Andrew H. Ragland; 770-873-6621$ 1,000 College JumpStart ScholarshipApplication Deadline: October 8thScholarship Description: The $ 1,000 College JumpStart Scholarship is a virtue-based competition that is open to 7th—12th graders and college students and non — traditional students. Applicants must be juniorsor seniors or adult students.Contact: Adrian Monk; 650-319-8441ACF Andrew Piech Memorial ScholarshipApplication Deadline: July 9thScholarship Description: One or more scholarships are awarded each year to New Mexico graduating high school seniors and continuing college students. Students must go after a degree or certificate from a non-profit public or technical professional institution including community college.Contact: Daniel White; 505-883-6240“Species On The Edge 2. 0” Social ScholarshipApplication Deadline: September 19thScholarship Description:Conserve Wildlife Foundation invites high school student from across the state to submit an original social media campaign showing why wildlife is important to protect. The fun and educational contest provides students with the opportunity to show their talent, creativity and love for nature. The students may get scholarships if they perform well.Contact: Stephanie Dalessio; 609-984-602121. What's the $ 1,000 College JumpStart Scholarship mainly based on?A. Certificate.B. Virtue.C. Protecting wildlife.D. Helping black students.22.Who can minority nationality students call for help if they want to get a scholarship?A. Stephanie Dalessio.B. Adrian Monk.C. Daniel White.D. Andrew H. Ragland.23. Which of the following is intended for New Mexican students?A. 5 Strong Scholarship.B. $ 1,000 College JumpStart Scholarship.C. ACF Andrew Piech Memorial Scholarship.D. “Species On The Edge 2. 0" Social Scholarship.BA bunch of strangers showed up at the gym in the early morning of the last Sunday of April. A few athletes were already stretching their arms, but most of us could barely focus. As I was burning off last night's wrong decision—a big meal, I spotted a poster about a gym's 21-day workout challenge, and I immediately signed up. I always wanted to train for a marathon, so I considered this as my warm-up. Besides, I needed to stop being lazy and this was my chance to make a change.It was easy to promise on paper, but three weeks of recommended exercise routines and diet restrictions wouldn't be easy. I stuck to working out 30 minutes a day, and I didn't disturb my normal routine. Instead, I had to be faced with the tough work of being more creative in my spare time, thus breaking some bad habits.Overcoming weaknesses with willpower was my goal for the next part of the journey. But the difficult part about making a challenge is realizing that “wanting" and “doing" are two very different things. Just like a career, you have to physically work for it rather than just wait for it to happen. But that's hard. Throughout the 21 days, I often told myself that the challenge was meaningless and tried to sabotage myself from exercising. However, finally I overcame that negative thought and kept working out.At the end of the challenge, I learned something even more important: Feeling the results is better than seeing them. I had fewer headaches and more energy, and was simply happier. I even felt a little smarter as I researched which foods were better for my body, and learned how to make healthier meals. I felt a sense of accomplishment, too, because even though I wanted to give up dozens of times, I didn't. I felt my waist got smaller and my arms got stronger, and I no longer felt guilty after having a piece of cake or a drink.24. What was the author's wrong decision?A. Going to the gym early.B. Training for a marathon.C. Eating a lot the previous night.D. Stopping being lazy for a change.25. What is paragraph 2 mainly about?A. Some of the author's bad habits.B. Some ways of the author's killing his spare time.C. The importance of sticking to the normal daily routine.D. The difficulty of the author's keeping on with the workout plan.26. What does the underlined word “sabotage" in paragraph 3 probably mean?A. Prevent.B. Hear.C. Improve.D. Distinguish.27. What did the author learn from the workout challenge?A. Eating healthy food is more important than exercising.B. Overcoming a challenge could bring good feelings to him.C. It's more important to see what he likes than just feel it.D. There was no need to feel guilty about things he disliked to do.CA new study shows that air pollution can cause a huge reduction in our intelligence. The study was a project involving researchers at Peking University in China and Yale University in the United States.The researchers reported that long-term exposure to air pollution can affect a person's mental abilities in two areas: Language and math.The researchers studied about 25,000 people from across China. Between 2010 and 2014, these Chinese men, women and children were given language and math tests. Then researchers compared the test results with measurements of pollution in the air, namely nitrogen dioxide and sulfur dioxide (二氧化硫).Xi Chen of the Yale School of Public Health led the study. He and his team found that breathing pollutedair can reduce a person's education level by about one year.Chen said that the effect generally is worse for those over 64 years of age, for men and for those with little or no education.“The older persons—they are more affected. And we find, quite amazingly, males are more affected than females. And people working outdoors are more affected than people working indoors.He noted that the youngest people in the study were 10 years old, while the oldest was 90 years old. They came from China's 33 provinces. In his words, the data and facts are convincing and this range of ages and locations across the country provided a real, objective and representative sample.The researchers noted that the effect of air pollution on verbal ability is even more serious as people age, especially among men and the less educated. The researchers also stressed that every country, whether developed, developing or poor, should focus on air pollution orhumans will face a bad situation.28. What is the result of the study?A. Air pollution has a bad effect on people's intelligence.B. Females are more affected by air pollution than males.C. Americans are more affected by air pollution than Chinese.D. People working indoors are actually not affected by air pollution.29. What did Chen say about the study?A. It is doubtful.B. It is extremely unfair.C. It is common and not representative.D. It is relatively objective and persuasive.30. What did the researchers emphasize in the last paragraph?A. The data from the 33 provinces is convincing.B. The whole world should pay attention to air pollution.C. The less educated take more responsibility for air pollution.D. Air pollution's influence on verbal ability is less serious as people age.31. Where is the text most likely taken from?A. A life diary.B. A guidebook.C. A science magazine.D. A biography.DStories are shared in many ways. They are described in books and magazines. They are read around the campfire at night. They are randomly distributed from stand-alone booths. But what else?To revive (复兴)literature in the era of fast news and smartphone addiction, Short Edition, a French publisher of short-form literature, has set up more than 30 story dispensers (分发机)in the USA in the past years to deliver fiction at the push of a button at restaurants, universities and government offices.Francis Ford Coppola, the film director and winemaker, liked the idea so much that he invested in the company and placed a dispenser at his Cafe Zoetrope in San Francisco. Last month public libraries in some other cities announced they would be setting them up, too. There is one on the campus at Penn State. A few can be found in downtown West Palm Beach, Fla. And Short Edition plans to announce more, including at the Los Angeles International Airport.“Everything old is n ew again," said Andrew Nurkin, the director of the Free Library of Philadelphia, which is one of the libraries that set up the dispensers. “We want people to be easily exposed to literature. We want to advance literacy among children and inspire theircreativity.Here's how a dispenser works: It has three buttons on top indicating choices for stories that can be read in one minute, three minutes or five minutes. When a button is pushed, a short story is printed. The stories are free. They are chosen from a computer category of more than 100,000 original submissions by writers whoseworks have been evaluated by Short Edition's judges, and transmitted over a mobile network. Offerings can be tailored to specific interests, like children's fiction or romance. Short Edition gets stories for its category by holding writing contests.Short Edition set up its first booth in 2016 and has 150 machines worldwide. “The idea is to make people happy," said Kristan Leroy, director of Short Edition, “There is too much unhappiness today. ”32. What do we know about the stories sent by dispensers?A. They are expensive.B. They are short in form.C. They can be read on smartphones.D. They are mainly taken from magazine literature.33. Where can you find the popularity of story dispensers in America?A. In paragraph 3.B. In paragraph 4.C. In paragraph 5.D. In paragraph 6.34. Which is the main purpose of setting up the dispensers according to Andrew Nurkin?A. To get rid of people's smartphone addiction.B. To reduce the financial stress of libraries.C. To make people have access to literature.D. To advertise the network literature.35. What is the best title for the text?A. Online Reading: a Virtual TourB. Short Edition, a French PublisherC. Everything Old Will Be Popular AgainD. Taste of Literature, at the Push of a Button七选五(福建省厦门外国语学校2020届高三12月月考)It is sometimes thought that the longing for material goods, the need to buy things, is a relatively modern invention. 36 Trade or shopping is certainly an ancient desire, and existed before our ancestors invented writing, laws, cities or farming, even before they used metal to make tools.Humans are born to trade. 37 Evidence from hunter-gatherers suggests that the exchange of food and other necessary things comes naturally, as well as the ability to keep a record of the credits involved. And once trade begins, the benefits are hard to resist.Ancient local coastal people in northern Australia traded fish hooks, along a chain of trading partners, with people living 400 miles inland, who cut and polished local stone to make axes(斧子). 38 Finally, both groups of "producers", by concentrating on things they could produce and exchanging them for other things they needed, benefited as a result.Trade in the necessities of life, such as food and simple tools, is not really surprising, considering the link between these basic items and survival. What is surprising, though, is that our taste for unnecessary expensive objects also goes back a long way.In South Africa, 100,000-year-old decorative dyes(染料)have been found in an area where none were produced. 39 Small round pieces of glass 76,000 years old were also found at the same place. The earliest jewellery known to us was not just random findings—they were grouped together in size and had holes like those used for threading onto a necklace.Archaeologists argue that trade prepared the way for the complex societies in which we live today. 40 However, their modern equivalents—fast cars and expensive clothes—hold the same attraction for us as "trade goods" did for people 100,000 years ago.A.And we don't need shops or money to do it.B.These are powerful proofs for cash purchase.C.In fact, its roots go back to the beginning of humanity.D.However, the first trade began from the exchange of objects.E.Modern-day shoppers may not be impressed by ancient glass pieces.F.It is thought that these goods were bought at least 30 kilometres away. G.Every individual along the chain made a profit, even if he produced neither himself.参考答案完形填空36-40 BABDA 41-45ADACD 46-50DBBCB 51-55DCBCD阅读理解21 -23 BDC 24 -27 CDAB 28 -31 ADBC 32-35 BACD七选五36-40 CAGFE。
2020普通高等学校招生全国统一考试(新高考模拟卷)

英语试题 第1页(共10页) 英语试题 第2页(共10页)绝密★启用前|全国一线试题命制中心2020年普通高等学校招生全国统一模拟【新课标4卷】英 语(考试时间:120分钟 试卷满分:150分)注意事项:1. 答卷前,考生务必将自己的姓名、准考证号填写在答题卡上。
2. 回答选择题时,选出每小题答案后,用铅笔把答题卡上对应题目的答案标号涂黑。
如需改动,用橡皮擦干净后,再选涂其他答案标号。
回答非选择题时,将答案写在答题卡上,写在本试卷上无效。
3. 考试结束后,将本试卷和答题卡一并交回。
第一部分 听力(共两节,满分30分)做题时,请先将答案标在试卷上。
录音内容结束后,你将有两分钟的时间将试卷上的答案转涂到答题卡上。
第一节 (共5小题;每小题1.5分,满分7.5分)听下面5段对话。
每段对话后有一个小题,从题中所给的A 、B 、C 三个选项中选出最佳选项。
听完每段对话后,你都有10秒钟的时间来回答有关小题和阅读下一小题。
每段对话仅读一遍。
例:How much is the shirt? A. £ 19.15. B. £ 9.18. C. £ 9.15.答案是 C 。
1.Why does the man want to leave? A .The service is too slow. B .The food is bad. C .The music is too loud. 2.What does the woman do? A .A teacher. B .A nurse. C .A shop assistant.3.What has the man decided to do? A .Continue his talk with Mr Black. B .Go to see an engineer. C .Check the schedule.4.Where does the conversation most probably take place?A .On a bus.B .In a library.C .In a shop.5.How did the man feel about his jump in the end? A .Terrified. B .Disappointed. C .Excited.第二节 (共15小题;每小题1.5分,满分22.5分)听下面5段对话或独白。
备考2020年高考新课标选填题常规训练套题理科(8套)

否是否是输出c 输出b输出ab ≥c?a ≥c?开始输入a,b,ca ≥b ?否是结束备考2020年高考新课标选填题常规训练套题理科(8套)高考选、填题训练(一)一、选择题:1.集合{4},{3}A x x B x x =|2≤<=|≥,则AB =A .[2,4)B .[3,)+∞C .[3,4)D .[2,3) 2.复数52i -的共扼复数是 A .2i + B .2i -+ C .2i - D .2i -- 3.“a b >”是“11a b<”成立的 A .充分不必要条件 B .必要不充分条件 C . 充要条件 D . 既不充分又不必要的条件 4.编号为1,2,3,4,5,6的六个同学排成一排,3、4号两位同学相邻,不同的排法 A .60种 B .120种 C .240种 D .480种 5.已知菱形ABCD 的对角线AC 长为4,则AD AC ⋅= A .2 B .4 C .6 D .86.设a ,b 为两条不同的直线,,αβ为两个不同的平面,则下列说法正确的是 A .若a ∥α,α⊥β,则a ∥β B . 若a ∥b ,a ∥β,则b ⊥αC .若a ∥α,b ∥α,则a ∥b D . 若a ⊥b ,a ∥α,则b ⊥α7.函数2()lg(1)cos f x x x =+-的零点个数为 A .1 B .2 C .3 D .48.某算法程序框图如图所示,若1323,3,log 32a b c ===,则输出的结果是 A .3a b c ++ B .a C .b D .c9.若抛物线22y px =的焦点与双曲线22122x y -=的右焦点重合,则p 的值为 A .2- B .2 C .4D .4-10.函数ln ||y x x =的图象大致是 ( )ABCD俯视图二、填空题: 11.二项式6(x 的展开式中的常数项是 12. 如图所示,一个空间几何体的正视图和侧视图都是边长为1的正方形,俯视图是一个直径为1的圆,那么这个几何体的表面积为 13.已知a ,b 是正数,且3ab a b =++,则ab 的最小值为14.过双曲线2212y x -=的右焦点作直线l 交双曲线于A 、B 两点,若满足AB λ||=的直线l 共有3条,则实数λ=15、已知函数⎩⎨⎧≥+-<=)0(4)3(),0()(x a x a x a x f x 满足对任意0)()(,212121<--≠x x x f x f x x 都有成立,则a 的取值范围是16、 如图,在ΔABC 中,0AH BC ⋅=,且AH=1,G 为ABC ∆的重心,则GH AH ⋅==高考选、填题训练(二)一、选择题:1.已知集合A ={x |(1)(5)0x x --<},B ={x |04x <≤},则集合A B =(A ){x | 0<x <4}(B ){x | 0<x <5} (C ){x | 1<x ≤ 4}(D ){x | 4≤x <5}2.()102x -的展开式中第5项的二项式系数是( )A 510CB 41610C C 43210C -D 410C3.下列说法正确的是(A )“(0)0f =”是“函数()f x 是奇函数”的充要条件(B )若0:p x ∃∈R ,2010x x -->,则:p ⌝x ∀∈R ,210x x --< (C )若p q ∧为假命题,则p ,q 均为假命题 (D )“若6πα=,则1sin 2α=”的否命题是“若6πα≠,则1sin 2α≠” 4.在一次对人体脂肪含量和年龄关系的研究中,研究人员获得了一组样本数据,并制作成如图所示的人体脂肪含量与年龄关系的散点图.根据该图,下列结论中正确的是(A )人体脂肪含量与年龄正相关,且脂肪含量的中位数等于20% (B )人体脂肪含量与年龄正相关,且脂肪含量的中位数小于20% (C )人体脂肪含量与年龄负相关,且脂肪含量的中位数等于20%(D )人体脂肪含量与年龄负相关,且脂肪含量的中位数小于20%5.如图,已知A ,B 两点分别在河的两岸,某测量者在点A 所在的河岸边另选定一点C ,测得50AC =m ,45ACB ∠=,105CAB ∠=,则A 、B 两点的距离为(A)m (B) (C) (D)6.已知不等式组,,y x y x x a ≤⎧⎪≥-⎨⎪≤⎩(其中0a >)表示的平面区域的面积为4,点(,)P x y 在该平面区域内,则2z x y=+的最大值为 (A )9(B )6 (C )4(D )37.已知函数2()24f x x x =-+在区间[0,]m (0m >)上的最大值为4,最小值为3,则实数m 的取值范围是 (A )[1,2](B )(0,1] (C )(0,2](D )[1,)+∞8.已知实数[1,10]x ∈,执行如右图所示的程序框图,则输出x 的值不小于55的概率为(A )19 (B )29 (C )49 (D )599. 已知1sin 23α=,则2cos ()4πα-=( ) A .13- B .23- C .13 D .2310.直线l :30x y +-=分别与函数3xy =和3log y x =的交点为11(,)A x y 、22(,)B x y ,则122()y y +=A .4B .6C .8D .不确定 二、填空题:11.已知tan 3α=,则3cos sin 2cos sin()ααααπ+=++______.12.在Rt △ABC 中,2C π=,6B π=,1CA =,则|2|AC AB -=_____.13.顶点在原点,对称轴是y 轴,并且经过点(4,2)P --的抛物线方程是__________.14.右图中的网格是边长为1的小正方形,在其上用粗线画出了某多面体的三视图,则该多面体的体积为__________.15.已知双曲线22221(0,0)x y a b a b -=>>的渐近线与圆22420x y x +-+=相切,则该双曲线的离心率是__________.附加:.已知8()x a +的展开式中5x 的系数是7-,则实数a =________.高考选、填题训练(三)一、选择题(本大题共10小题,每小题5分,共50分.)1.设复数i i z (1--=是虚数单位),z 的共轭复数为-z ,则=⋅--z z )1(( )A .10B .2C .2D .12.已知集合{}{}b a B A a ,,2,1==,若⎭⎬⎫⎩⎨⎧=21B A ,则B A 为()A 、⎭⎬⎫⎩⎨⎧b ,1,21B 、⎭⎬⎫⎩⎨⎧-21,1 C 、⎭⎬⎫⎩⎨⎧1,21 D 、⎭⎬⎫⎩⎨⎧-1,21,13.已知直线a 和平面βα,,βαβα⊄⊄=a a l ,, ,且a 在βα,内的射影分别为直线c b ,,则直线c b ,的位置关系为( )A .相交或平行B .相交或异面C .平行或异面D .相交、平行或异面4.对任意非零实数b a ,,若b a ⊗的运算规则如下图的程序框图所示,则4)23(⊗⊗的值是( ) A .0 B .21 C .23D .9 5. }{n a 为各项都是正数的等比数列,n S 为前n 项和,且70,103010==S S ,那么=40S ( ) A .150 B .200- C .150或200- D .400或50- 6、已知函数1cos 22sin )(2-+=x x x f ,将)(x f 的图像上各点的横坐标缩短为原来的21倍,纵坐标不变,再将所得图像向右平移4π个单位,得到函数)(x g y =的图像。
2020年普通高等学校全国统一招生考试(江苏卷)预测卷数学试题及答案

绝密★启用前2020年普通高等学校全国统一招生考试(江苏卷)预测卷数学I注意事项:1、答题前填写好自己的姓名、班级、考号等信息 2、请将答案正确填写在答题卡上一、填空题:本大题共14小题,每小题5分,共70分.请把答案直接填写在答题卡相应位置上.1.已知集合A={x|x<0},B={-2,-1,0,2},则A ∩B=___2.已知复数z 满足112z i i=++(i 是虚数单位),则复数z 的共轭复数为___ 3.某地区有小学生、初中生、高中生的人数见下表.采用分层抽样的方法调查学生的眼睛视力状况,在抽取的样本中初中生有320人,则该样本中的高中生人数为_____类别小学生 初中生 高中生 合计 人数 18000 16000 9000 430004.5.函数2()ln )(9f x x =-的定义域为____6.有3名学生甲、乙、丙,在分发数学作业时,从他们3人作业中各随机取出1份作业,则这3名学生恰好都拿到自己作业的概率为_____7.已知等比数列{}n a 满足11,2a =且2434(1),a a a =-则5a =____ 8.已知f(x)是定义在R 上的奇函数,当x>0时,2()log 3f x x =-,则f(f(-16))的值为_____9.某品牌汽车4S 店一年销售汽车4000辆,每次从汽车公司购置x 辆,运费为4万元/次,一年的总储存费用为0.4x 万元.要使一年的总运费与总储存费用之和最小的,则x 的值为_____.10.在平面直角坐标系xOy 中,已知抛物线220y px p =>的准线1与双曲线2221x y a a-=>0的两条渐近线围成等边三角形,且面积为3,则p+a=_____. 11.如图,在正四棱柱形容器内盛有水和相同高度的实心圆柱(其中圆柱底面内切于正四棱柱底面,水面恰与正四棱柱上底面齐平),将实心圆柱拿去后,则水面高度与正四棱高度比为____.(不计水的损耗)12.如图,△ABC 中,M 为AB 中点,AB=5,CM=3,EF 为圆心为C,半径为1的圆的动直径,则BE AF ⋅的取值范围是_____13.在平面直角坐标系xOy 中,圆221:4O x y +=与圆2222:(4)(0)O x y r r -+=>,在圆2O 上存在点Q,过点Q 作圆1O 的切线,切点为P,N,使得5,9QP QN ⋅=则实数r 的最小值为___. 14.已知函数3,1,(),1,x a x f x x ax x -≥⎧=⎨-<⎩若函数y=f[f(x)]恰有5个不同零点,则实数a 的取值范围是____. 二、解答题:本大题共6小题,共计90分.请在答题卡指定区域内作答.解答时应写出文字说明、证明过程或演算步骤.15.(本小题满分14分)在平面直角坐标系xOy 中,以x 轴正半轴为始边作两个钝角α,β,它们的终边分别与单位圆交于A,B 两点.已知A,B 的横坐标分别为3102,.1010-- 求:(1)cos(α-β)的值;(2)2α-β的值.16.(本小题满分14分)如图,三棱锥P-ABC 中,已知PA ⊥底面ABC,AC ⊥BC,且PA=AC,点E,F 分别是棱PC,PB 的中点.(1)求证:AE ⊥BC;(2)点G 为棱AB 上一点,满足2,GB GA =求证:AE//平面CFG.17.(本小题满分14分)如图,在平面直角坐标系xOy 中,椭圆22221(0),x y a b a b +=>>圆C:222().4b x y b +-=A,B 分别为椭圆的左、右顶点,直线AC 交圆C 于D,P 两点(D 在线段AC 上),且2.AD DC =(1)求椭圆的离心率;(2)直线BP 与椭圆相交于点Q,直线AQ 被圆C 截得弦长为6,3求椭圆的标准方程. 18.(本小题满分16分)如图为某野生动物园一角,∠MOK 内区域为陆地动物活动区,∠NOK 内区域为水上动物活动区.为满足游客游览需要,现欲在OM,ON 上分别选一处A,B,修建一条贯穿两区域的直路AB,AB 与KO 相交于点P.若PA 段,PB 段每百米修路费用分别为1万元和2万元,已知∠NOK=30°,OM⊥OK,OP=2百米,设∠PAO=α.(1)试将修路费用表示为α的函数()S α(2)求修路费用()S α的最小值.19.(本小题满分16分)设等差数列{}n a 的前n 项和为,n S 且7146,54.a a S == (1)求数列{}n a 的通项公式;(2)是否存在正整数m,k,使得31,1,1m m k a a a +---依次成等比数列?并说明理由; (3)设数列{}n b 满足2*1()(),5n n a b n -=∈N 将{}n a 和{}n b 中相同的项按照从小到大的顺序依次排列,得到数列{},n c 求数列{}n c 的通项公式.20.(本小题满分16分)已知函数y=f(x)的定义域为D,若满足∀x ∈D,(x-1)f(x)≥0,则称函数f(x)为“L 型函数”.(1)判断函数xy e =和y=lnx 是否为“L 型函数”,并说明理由;(2)设函数f(x)=(x+1)lnx-(x-1)lna(a>0),记()().g x f x '=①若函数g(x)的最小值为1,求a 的值;②若函数f(x)为“L 型函数”,求a 的取值范围.21.[选做题]本题包括A 、B 、C 三小题,请选定其中两题,并在相应的答题区域内作答.若多做,则按作答的前两题评分.解答时应写出文字说明、证明过程或演算步骤.A.选修4-2:矩阵与变换(本小题满分10分) 已知矩阵记1040,10102A B ⎢⎥⎡⎤⎢⎥==⎢⎥⎢⎥⎣⎦⎣⎦记M=AB,求1.M B.选修4-4:坐标系与参数方程(本小题满分10分)在平面直角坐标系xOy 中,已知直线1,12x l y l=-+⎧⎨=-+⎩(l 为参数)与曲线cos ,cos 2x y θθ=⎧⎨=-⎩(θ为参数)的交点为A,B,求线段AB 的长.C.选修4-5:不等式选讲(本小题满分10分)已知x,y,z 均是正实数,且2229436.x y z ++=,求证:x+y+z ≤7.[必做题]第22、23题,每小题10分,共计20分.请在答题卡指定区域内作答,解答时应写出文字说明、证明过程或演算步骤.22.(本小题满分10分)如图1,某电视台一档综艺节目的游戏挑战项目“蜂巢迷宫”的道具,游戏规定挑战者必须“蒙眼”进行现简化模型如图2所示,共有A,B,C,D,E,F 六个房间组成,每个房间各有六扇门分别与相邻房间或与外部相通,假设打开每扇门都是等可能的.现挑战者从房间A 出发,要求到达房间E.(1)求挑战者“打开两扇门完成挑战”的概率;(2)一次游戏中规定“只要走出道具外部或打开超过四扇门(含四扇)挑战失败”,得0分;“打开三扇门完成挑战”,得1分,“打开两扇门完成挑战”,得2分.挑战者共挑战1次,得分设为X,求随机变量X 的概率分布和数学期望E(X).23.(本小题满分10分)(1)用数学归纳法证明二项式定理:011()n n n n n a b C a C a b -+=++222*,.n r n r r n n n n n C a b C a b C b n --++++∈N(2)利用二项式定理求证:220()n k n n n k CC ==∑。
2020年普通高等学校招生全国统一考试新高考数学押题密卷
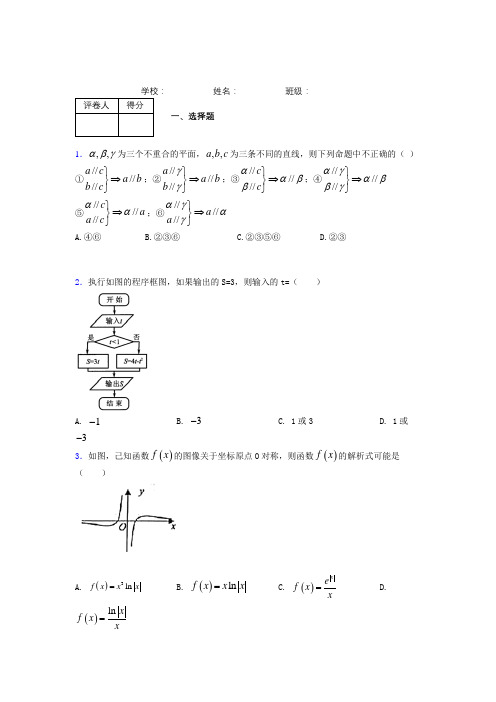
__________ 姓名:__________ 班级:__________评卷人 得分一、选择题1.γβα,,为三个不重合的平面,c b a ,,为三条不同的直线,则下列命题中不正确的( )①b a c b c a //////⇒⎭⎬⎫;②b a b a //////⇒⎭⎬⎫γγ;③βαβα//////⇒⎭⎬⎫c c ;④βαγβγα//////⇒⎭⎬⎫ ⑤a c a c //////αα⇒⎭⎬⎫;⑥αγγα//////a a ⇒⎭⎬⎫ A.④⑥ B.②③⑥ C.②③⑤⑥ D.②③2.执行如图的程序框图,如果输出的S=3,则输入的t=( )A. 1-B. 3-C. 1或3D. 1或3-3.如图,己知函数()f x 的图像关于坐标原点O 对称,则函数()f x 的解析式可能是( )A. ()3ln f x x x =B. ()ln f x x x =C. ()xef x x=D.()ln xf x x=4.在平面直角坐标系中,角α的终边与单位圆交于点34(,)55P -,则sin()4πα+=( )A.22221:4AA A AC C C Cv a r v v a v r ===B. -10C.10D. -105.在平行四边形ABCD 中,E,F 分别为边BC,CD 的中点,若AB x AE y AF =+(,),x y R ∈则 x y += ( ) A. 2B. 1C.32D.236.已知O 为四边形ABCD 所在的平面内的一点,且向量OA ,OB ,OC ,OD 满足等式OA OC OB OD +=+,若点E 为AC 的中点,则EABBCDS S ∆∆=( ) A.14B.12 C.13D.237.已知实数x ,y 满足2210y x y x y ≤⎧⎪+≥⎨⎪-+≤⎩,则3x +2y 的最大值为A.7B.5C.4D.928.解三角方程时尤其要注意角度的取值范围.9.定义在R 上的函数f (x )满足f (x +y )=f (x )+f (y )+2xy (x ,y ∈R),f (1)=2,则 f (-3)等于 ( ) A .2 B .3 C .6D .9二、填空题10.已知点A 是抛物线214y x =的对称轴与准线的交点,点F 为该抛物线的焦点,点P 在抛物线上且满足PF m PA =,则m 的最小值为 .评卷人 得分三、解答题11.(1)解关于x 不等式2111x x-≤- (2)若函数2()6(8)f x kx kx k =-++的定义域为R ,求实数k 的取值范围。
最新2020年高考【文科数学】填空题专练20套Word版含答案及解析

最新2020年高考【文科数学】填空题专练20套最新2020年高考【文科数学】填空题专练(01)二、填空题:本题共4小题,每小题5分,共20分.13.已知向量与互相垂直,则=_______.【答案】1【解析】【分析】向量与互相垂直,可得,即可得出结果.【详解】∵向量与互相垂直,∴,解得,故答案为1.【点睛】本题主要考查了向量垂直与数量积的关系,考查了推理能力与计算能力,属于基础题.14.已知实数满足约束条件,则的最大值为________.【答案】3【解析】【分析】作出不等式组对应的平面区域,设,利用数形结合求得最优解,计算最大值.【详解】作出不等式组所表示的区域如图:,为目标函数,可看成是直线的纵截距,画直线,平移直线过点时有最大值3,由得,即点坐标为故的最大值为,故答案为3.【点睛】本题主要考查线性规划中利用可行域求目标函数的最值,属简单题.求目标函数最值的一般步骤是“一画、二移、三求”:(1)作出可行域(一定要注意是实线还是虚线);(2)找到目标函数对应的最优解对应点(在可行域内平移变形后的目标函数,最先通过或最后通过的顶点就是最优解);(3)将最优解坐标代入目标函数求出最值.15.已知函数,则函数在的值域为______.【答案】【解析】【分析】利用三角恒等变换化简函数的解析式,再利用余弦函数的定义域和值域,求得函数在的值域.【详解】∵函数,在上,,,,故答案为.【点睛】本题主要考查三角恒等变换,余弦函数的定义域和值域,解题的关键在于将函数式化为三角函数的基本形式,属于中档题.16.双曲线C:的左、右焦点为F1,F2,直线与C的右支相交于点P,若,则双曲线C的离心率为______.【答案】【解析】联立直线与双曲线方程求出的坐标,利用双曲线的定义,转化求解双曲线的离心率即可.【详解】把代入的方程可得,∴,,,由双曲线的定义可知:,,∴,整理可得,∴,所以双曲线的离心率为.故答案为.【点睛】本题主要考查了双曲线的简单性质的应用,常见的离心率的求法有:1、直接求出,求解;2、变用公式(双曲线),(椭圆);3、构造关于的齐次式解出等,属于中档题.最新2020年高考【文科数学】填空题专练(02)二、填空题:本题共4小题,每小题5分,共20分.13.已知点,线段的中点,若向量与向量垂直,则_____.【答案】【解析】【分析】根据条件可求出,根据即可得出,进行数量积的坐标运算即可求出.【详解】;∵;∴;∴.故答案为:.【点睛】向量的数量积有两个应用:(1)计算长度或模长,通过用;(2)计算角,.特别地,两个非零向量垂直的充要条件是.14.如图,在边长为2的正方形中,以的中点为圆心,以为半径作圆弧,交边于点,从正方形中任取一点,则该点落在扇形中的概率为_____.【答案】【解析】【分析】由已知求出扇形面积与正方形面积,再由测度比是面积比得所求概率.【详解】如图,正方形面积,因,故,所以,同理,所以,又,∴.∴从正方形中任取一点,则该点落在扇形中的概率为.故答案为:.【点睛】本题考查几何概型,求出扇形面积是关键,是基础题.15.在中,,,,则_________.【答案】【解析】【分析】根据题意,由正弦定理可得,即,变形可得,又由,结合二倍角公式可得,变形可得:,,进而求出和的值,又由,由和角公式计算可得答案.【详解】根据题意,中,,则,即,变形可得,又由,即,则有,变形可得:,则,则,,则,故答案为:.【点睛】三角形中共有七个几何量(三边三角以及外接圆的半径),一般地,知道其中的三个量(除三个角外),可以求得其余的四个量.(1)如果知道三边或两边及其夹角,用余弦定理;(2)如果知道两边即一边所对的角,用正弦定理(也可以用余弦定理求第三条边);(3)如果知道两角及一边,用正弦定理.16.已知函数.若存在,使得,则实数的取值范围是_________.【答案】【解析】【分析】求原函数的导函数,代入,得到存在,使得,分离参数,再由函数单调性求最值得答案.【详解】∵,∴,∴,∵存在,使得即,∴在上有解,设,∴,在上为增函数,∴.∴.实数的取值范围是.【点睛】含参数的不等式的有解问题,优先考虑参变分离的方法,把问题归结为不含参数的函数的值域问题.在转化过程中注意等价变形.最新2020年高考【文科数学】填空题专练(03)二、填空题:本大题共4小题,每小题5分,共20分.13.函数则__________.【答案】1.【解析】【分析】根据分段函数的解析式逐步代入求解可得结果.【详解】由题意得.故答案为:1.【点睛】本题考查分段函数的求值,解题时根据函数中不同的范围对应的解析式求解,属于简单题.14.已知,是第二象限的角,则__________.【答案】.【解析】【分析】由求得,再根据平方关系得到,于可得所求.【详解】∵,又是第二象限的角,,∴,∴,∴.【点睛】解题中在运用平方关系求值时要注意所求值的符号,本题考查同角三角函数关系式,属于简单题.15.已知的面积为,且,则__________.【答案】.【解析】由的面积为可得,再由可得,然后根据以上两式得到,由此可得.【详解】∵的面积为,∴①.∵,∴②.由①②两式得,又,∴.故答案为:.16.在《九章算术》中有称为“羡除”的五面体体积的求法.现有一个类似于“羡除”的有三条棱互相平行的五面体,其三视图如图所示,则该五面体的体积为__________.【答案】24.【解析】【分析】由三视图得到五面体的直观图,然后根据几何体的结构特征,利用分割的方法求得其体积.【详解】由三视图可得,该几何体为如下图所示的五面体,其中,底面为直角三角形,且,侧棱与底面垂直,且.过点作,交分别于,则棱柱为直棱柱,四棱锥的底面为矩形,高为.所以.故答案为:.【点睛】本题考查三视图还原几何体和不规则几何体体积的求法,考查空间想象能力和计算能力,解题的关键是由三视图得到几何体的直观图,属于基础题.最新2020年高考【文科数学】填空题专练(04)二、填空题:本大题共4小题,每小题5分,共20分.13.某校高三科创班共48人,班主任为了解学生高考前的心理状况,将学生按1至48的学号用系统抽样方法抽取8人进行调查,若抽到的最大学号为48,则抽到的最小学号为______.【答案】6【解析】【分析】抽到的最大学号为48,由系统抽样等基础知识即可得最小学号.【详解】由系统抽样方法从学号为1到48的48名学生中抽取8名学生进行调查,把48人分成8组,抽到的最大学号为48,它是第8组的最后一名,则抽到的最小学号为第一组的最后一名6号.故答案为:6.【点睛】本题考查了系统抽样等基础知识,考查运算求解能力,属于基础题.14.平面向量与的夹角为,,,则__________.【答案】【解析】【分析】由平面向量模的计算公式,直接计算即可.【详解】因为平面向量与的夹角为,所以,所以;故答案【点睛】本题主要考查平面向量模的计算,只需先求出向量的数量积,进而即可求出结果,属于基础题型.15.如图所示,正方体的棱长为2,E,F为,AB的中点,M点是正方形内的动点,若平面,则M点的轨迹长度为______.【答案】【解析】取的中点,的中点,连接,,,可得:四边形是平行四边形,可得.同理可得可得面面平行,进而得出点轨迹.【详解】如图所示,取的中点,的中点,连接,,,.可得:四边形是平行四边形,.同理可得:..平面平面,点是正方形内的动点,若平面.点在线段上.点的轨迹长度.故答案为.【点睛】本题考查了面面平行的判定定理与线面平行的判断,属于中档题.证明线面平行的常用方法:①利用线面平行的判定定理,使用这个定理的关键是设法在平面内找到一条与已知直线平行的直线,可利用几何体的特征,合理利用中位线定理、线面平行的性质或者构造平行四边形、寻找比例式证明两直线平行.②利用面面平行的性质,即两平面平行,在其中一平面内的直线平行于另一平面.16.在中,内角所对的边分别为,是的中点,若且,则面积的最大值是___【答案】【解析】【分析】由题意及正弦定理得到,于是可得,;然后在和中分别由余弦定理及可得.在此基础上可得,再由基本不等式得到,于是可得三角形面积的最大值.【详解】如图,设,则,在和中,分别由余弦定理可得,两式相加,整理得,∴.①由及正弦定理得,整理得,②由余弦定理的推论可得,所以.把①代入②整理得,又,当且仅当时等号成立,所以,故得.所以.即面积的最大值是.故答案为.【点睛】本题考查解三角形在平面几何中的应用,解题时注意几何图形性质的合理利用.对于三角形中的最值问题,求解时一般要用到基本不定式,运用时不要忽视等号成立的条件.本题综合性较强,考查运用知识解决问题的能力和计算能力.最新2020年高考【文科数学】填空题专练(05)二、填空题:本大题共4小题,每小题5分,共20分.13.已知向量、满足,,且,则与的夹角为________.【答案】.【解析】【分析】根据即可得到,进行数量积的运算便可求出,从而求出向量的夹角.【详解】解:∵;∴=0;∴;∵与夹角的取值范围为[0,π],∴的夹角为.故答案为:.【点睛】本题考查向量垂直的充要条件,向量数量积的运算及计算公式,以及向量夹角的范围.14.的三个内角,,所对的边分别为,,,为的中点,,,且,则________.【答案】.【解析】【分析】由已知利用正弦定理,三角函数恒等变换的应用可求cos C,可得C=60°,在平行四边形ACBD,可求∠CAD=120°,,由余弦定理可得a,利用三角形面积公式即可得解.【详解】解:2c cos B=2a﹣b⇒2sin C cos B=2sin A﹣sin B⇒2sin C cos B=2sin B cos C+2cos B sin C﹣sin B⇒cos C,所以C=60°.如图补成平行四边形ACBD,则∠CAD=120°,,在△ADC中,由余弦定理得:,所以:,故答案为:【点睛】本题主要考查了正弦定理,三角函数恒等变换的应用,余弦定理,三角形面积公式,平面向量数量积的运算在解三角形中的综合应用,考查了运算求解能力和转化思想,属于中档题.15.已知是抛物线的焦点,是上一点,直线交直线于点.若,则________.【答案】8【解析】如图,记直线与y轴的交点为N,过点P作与M,因为,所以,所以又因为,所以,故. 故答案为:8.点睛:求解解析几何中的问题,包括几何法和代数法,如几何法经常涉及圆锥曲线的定义和比较明显的平面几何的定理和性质,所以做题时要充分考虑这些定义来进行转化,比如椭圆和双曲线定义涉及两条焦半径,所以给出,就联想 ,抛物线有,就联想到准线的距离.16.已知半径分别为1和2的两球紧贴放在水平桌面上,则两球在桌面上的俯视图的公共弦长为________.【答案】.【解析】可求出两球的球心距离为1+2=3,两球的球心的垂直距离为2﹣1=1,水平距离为2,作出两球在桌面上的俯视图,运用解三角形的知识和等积法,即可得到所求值.【详解】解:半径分别为1和2的两球紧贴放在水平桌面上,可得两球的球心距离为1+2=3,两球的球心的垂直距离为2﹣1=1,水平距离为2,两球在桌面上的俯视图如右图:且AO1=1,AO2=2,O1O2=2,cos∠O1AO2,则sin∠O1AO2,△AO1O2的面积为S1×2,可得O1O2上的高为,则两球在桌面上的俯视图的公共弦长为2.故答案为:.【点睛】本题考查球的投影和两圆的位置关系和弦长求法,考查空间想象能力和运算能力,属于中档题.最新2020年高考【文科数学】填空题专练(06)二、填空题:本大题共4小题,每小题5分,共20分.13.若函数()ln f x x a x =-在点(1,1)处的切线方程为21y x =-,则实数a =_________. 【答案】-1 【解析】 【分析】利用导数的几何意义求出曲线在点()1,1处的切线斜率为12a -=,从而可得结果. 【详解】因为函数()ln f x x a x =-的导数为()1af x x'=-, 所以在点()1,1处的切线斜率为()'11f a =-, 又因为在点()1,1处的切线方程为21y x =-, 所以12a -=,解得1a =-,故答案为1-.【点睛】本题主要考查利用导数求切线斜率,属于基础题. 应用导数的几何意义求切点处切线的斜率,主要体现在以下几个方面:(1) 已知切点()()00,A x f x 求斜率k ,即求该点处的导数()0k f x '=;(2) 己知斜率k 求参数或切点()()11,,A x f x 即解方程()1f x k '=;(3) 巳知切线过某点()()11,M x f x (不是切点) 求切点, 设出切点()()00,,A x f x 利用()()()10010f x f x k f x x x -'==-求解.14.执行如图所示的程序框图,输出的S 为_________.【答案】1 【解析】【分析】模拟执行程序框图,只要按照程序框图规定的运算方法逐次计算,直到达到输出条件即可得到输出的S 的值.【详解】执行程序框图,输入0,1==S n , 第一次循环1,2S n ==; 第二次循环1,3S n ==; 第三次循环0,4S n ==; 第四次循环0,5S n ==; 第五次循环1,6S n ==; 第六次循环1,7S n ==; 第七次循环0,8S n ==; 第八次循环0,9S n ==; 第九次循环1,10S n ==; 第十次循环1,11S n ==; 退出循环输出1S =,故答案为1.【点睛】本题主要考查程序框图的循环结构流程图,属于中档题. 解决程序框图问题时一定注意以下几点:(1) 不要混淆处理框和输入框;(2) 注意区分程序框图是条件分支结构还是循环结构;(3) 注意区分当型循环结构和直到型循环结构;(4) 处理循环结构的问题时一定要正确控制循环次数;(5) 要注意各个框的顺序,(6)在给出程序框图求解输出结果的试题中只要按照程序框图规定的运算方法逐次计算,直到达到输出条件即可.15.已知向量a r ,b r,1a =r ,(2,1)b =r ,且0(0)a b λλ+=>r r ,则λ=_________.【解析】 【分析】设a =r (x ,y ).由于向量a r ,b r 满足|a r |=1,b =r (2,1),且0a b λ+=r r r (λ∈R ),可得12010x y λλ=+=⎨⎪+=⎪⎩,解出即可. 【详解】设a =r(x ,y ).∵向量a r ,b r 满足|a r |=1,b =r (2,1),且0a b λ+=r r r (λ∈R ),∴a b λ+=rrλ(x ,y )+(2,1)=(λx +2,λy +1),∴12010x y λλ=+=⎨⎪+=⎪⎩,化为λ2=5.解得λ=【点睛】本题考查了向量的坐标运算、向量的模的计算公式、零向量等基础知识与基本技能方法,属于基础题.16.已知双曲线C :22221(0,0)x y a b a b-=>>的右焦点为F ,左顶点为A ,以F 为圆心,FA为半径的圆交C 的右支于M ,N 两点,且线段AM 的垂直平分线经过点N ,则C 的离心率为_________. 【答案】43【解析】 【分析】先证明AMN ∆是正三角形,在'MFF ∆中,由余弦定理、结合双曲线的定义可得2222||2||cos120(||2)FF FM FF FM F M FM a ''︒'+-==+,化为22340c ac a --=,从而可得结果.【详解】由题意,得()(,0),,0A a F c -,另一个焦点(),0F c '-,由对称性知,AM AN =,又因为线段AM 的垂直平分线经过点N ,, 则AN MN =,可得AMN ∆是正三角形, 如图所示,连接MF ,则AF MF a c ==+, 由图象的对称性可知,1302MAF NAF MAN ︒∠=∠=∠=, 又因为AMF ∆是等腰三角形, 则120AFM ︒∠=, 在'MFF ∆中,由余弦定理:2222||2||cos120(||2)FF FM FF FM F M FM a ''︒'+-==+,上式可化为22214()22()(3)2c a c c a c a c ⎛⎫++-⨯+-=+ ⎪⎝⎭, 整理得:22340c ac a --=,即()()34=0c a c a +-,由于0,0a c >>, 则4340,3c a c a -==, 故43c e a ==,故答案为43.【点睛】本题主要考查利用双曲线的简单性质求双曲线的离心率,属于中档题.求解与双曲线性质有关的问题时要结合图形进行分析,既使不画出图形,思考时也要联想到图形,当涉及顶点、焦点、实轴、虚轴、渐近线等双曲线的基本量时,要理清它们之间的关系,挖掘出它们之间的内在联系.求离心率问题应先将 e 用有关的一些量表示出来,再利用其中的一些关系构造出关于e 的等式,从而求出e 的值.本题是利用点到直线的距离等于圆半径构造出关于e 的等式,最后解出e 的值.最新2020年高考【文科数学】填空题专练(07)二、填空题. 13.已知函数的最小正周期为,则=_____,若,则=____.【答案】 (1). 2 (2).【解析】由题意利用正弦函数的周期性求得ω,再利用同角三角函数的基本关系,二倍角公式,求得sin2α的值.【详解】由周期公式,可得ω=2,由,得,所以,平方得,∴故答案为:2;.【点睛】本题主要考查正弦函数的周期性,同角三角函数的基本关系,二倍角公式的应用,属于基础题.14.已知矩形,以为焦点,且过两点的双曲线的离心率为_______.【答案】【解析】【分析】根据为焦点,得;又求得,从而得到离心率.【详解】为焦点在双曲线上,则又15.我国古代数学名著《九章算术•商功》中阐述:“斜解立方,得两堑堵.斜解堑堵,其一为阳马,一为鳖臑.阳马居二,鳖臑居一,不易之率也.合两鳖臑三而一,验之以棊,其形露矣.”若称为“阳马”的某几何体的三视图如图所示,图中网格纸上小正方形的边长为1,对该几何体有如下描述:①四个侧面都是直角三角形;②最长的侧棱长为;③四个侧面中有三个侧面是全等的直角三角形;④外接球的表面积为24π.其中正确的描述为____.【答案】①②④【解析】由三视图还原几何体,可知该几何体为四棱锥,PA⊥底面ABCD,PA=2,底面ABCD为矩形,AB=2,BC=4,然后逐一分析四个命题得答案.【详解】由三视图还原原几何体如图,可知该几何体为四棱锥,PA⊥底面ABCD,PA=2,底面ABCD为矩形,AB=2,BC=4,则四个侧面是直角三角形,故①正确;最长棱为PC,长度为2,故②正确;由已知可得,PB=2,PC=2,PD=2,则四个侧面均不全等,故③错误;把四棱锥补形为长方体,则其外接球半径为PC=,其表面积为4π×=24π,故④正确.∴其中正确的命题是①②④.故答案为:①②④.【点睛】本题考查由三视图还原原几何体,考查多面体外接球表面积与体积的求法,是中档题.16.已知数列中,,则=_____【答案】【解析】【分析】根据递推公式,可配凑出,从而得到为等差数列,通过求解前项和求得结果.【详解】可知:数列为等差数列,首项为,公差本题正确结果:【点睛】本题考查利用数列递推公式求解数列前项和问题,关键是能够采用倒数法,将递推公式整理为等差数列定义式形式,从而配凑出等差数列,利用等差数列相关知识求解得到结果.最新2020年高考【文科数学】填空题专练(08)二、填空题:本大题4小题,每小题5分,共20分,答案写在答题卡相应横线上。
2020普通高等学校招生全国统一考试(新高考模拟卷)

绝密★启用前|全国一线试题命制中心普通高等学校招生全国统一模拟【新课标4卷】英语(考试时间:120分钟试卷满分:150分)注意事项:1. 答卷前,考生务必将自己的姓名、准考证号填写在答题卡上。
2. 回答选择题时,选出每小题答案后,用铅笔把答题卡上对应题目的答案标号涂黑。
如需改动,用橡皮擦干净后,再选涂其他答案标号。
回答非选择题时,将答案写在答题卡上,写在本试卷上无效。
3. 考试结束后,将本试卷和答题卡一并交回。
第一部分听力(共两节,满分30分)做题时,请先将答案标在试卷上。
录音内容结束后,你将有两分钟的时间将试卷上的答案转涂到答题卡上。
第一节(共5小题;每小题 1.5分,满分7.5分)听下面5段对话。
每段对话后有一个小题,从题中所给的A、B、C三个选项中选出最佳选项。
听完每段对话后,你都有10秒钟的时间来回答有关小题和阅读下一小题。
每段对话仅读一遍。
例:How much is the shirt?A. £ 19.15.B. £9.18.C. £9.15.答案是C。
1.Why does the man want to leave?A.The service is too slow.B.The food is bad.C.The music is too loud.2.What does the woman do?A.A teacher.B.A nurse.C.A shop assistant.3.What has the man decided to do?A.Continue his talk with Mr Black.B.Go to see an engineer.C.Check the schedule.4.Where does the conversation most probably take place?A.On a bus. B.In a library.C.In a shop.5.How did the man feel about his jump in the end?A.Terrified.B.Disappointed.C.Excited.第二节(共15小题;每小题 1.5分,满分22.5分)听下面5段对话或独白。
2020年普通高等学校招生全国统一考试新高考数学押题密卷 (2)
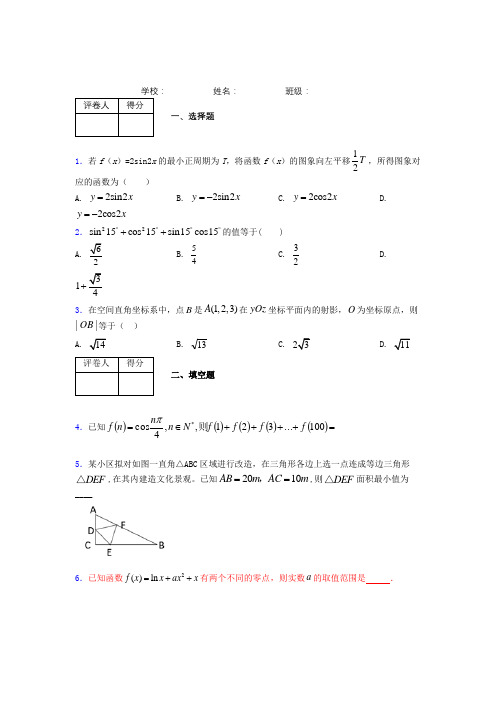
__________ 姓名:__________ 班级:__________评卷人 得分一、选择题1.若f (x )=2sin2x 的最小正周期为T ,将函数f (x )的图象向左平移12T ,所得图象对应的函数为( ) A. 2sin2y x =B. 2sin2y x =-C. 2cos2y x =D.2cos2y x =-2.22sin 15cos 15sin15cos15︒︒︒︒++的值等于( ) A.6 B.54C.23 D.314+3.在空间直角坐标系中,点B 是(1,2,3)A 在yOz 坐标平面内的射影,O 为坐标原点,则||OB 等于( )A. 14B. 13C. 23D. 11评卷人 得分二、填空题4.已知()()()()()=++++∈=*100...321,,4cosf f f f N n n n f 则π__________5.某小区拟对如图一直角△ABC 区域进行改造,在三角形各边上选一点连成等边三角形DEF △,在其内建造文化景观。
已知2010AB m AC m ==,,则DEF △面积最小值为____6.已知函数2()ln f x x ax x =++有两个不同的零点,则实数a 的取值范围是 .7.己知A(3,2),B(-2,1) ,若点P 在x 轴上,且有OP AB OB λ−−→−−→−−→=+(O 为平面直角坐标系的原点),则λ的值为评卷人 得分三、解答题8.(本小题满分12分)(2019·泉州质检)已知函数f (x )=x e x -a2x 2-ax . (1)讨论f (x )的单调性;(2)当x ≥-1时,f (x )+a2x 2-a +1≥0,求a 的取值范围. 9.如图,A ,B 两点相距2千米,6BAC π∠=.甲从A 点以v 千米/小时的速度沿AC 方向匀速直线行驶,同一时刻乙出发,经过t 小时与甲相遇.(1)若v = 12千米/小时,乙从B 处出发匀速直线追赶,为保证在15分钟内(含15分钟)能与甲相遇,试求乙速度的最小值;(2)若乙先从A 处沿射线AB 方向以16千米/小时匀速行进m (0<m <t )小时后,再以8千米/小时的速度追赶甲,试求甲在能与乙相遇的条件下v 的最大值.10.在10件产品中,有3件一等品,4件二等品,3件三等品.从这10件产品中任取3件.求:(1)取出的3件产品中一等品件数X 的分布列;(2)取出的3件产品中一等品件数多于二等品件数的概率.11.已知圆221:420C x y x y +-+=与圆222:240C x y y +--=.(1)求两圆公共弦所在直线的方程;(2)求过两圆的交点且圆心在直线241x y +=上的圆的方程. 12.(本小题10分)解下列不等式: (1) 423≤+x (2)1132≤+-x x【参考答案】***试卷处理标记,请不要删除一、选择题1.B 解析:B 【解析】 【分析】由三角函数的周期的公式得:T=22ππ=,由函数图象的平移得:g (x )=2sin2(x+2π)=-2sin2x ,得解.【详解】由f (x )=2sin2x 可得:此函数的最小正周期为T=22ππ=, 将函数f (x )的图象向左平移12T , 所得图象对应的函数为g (x )=2sin2(x+2π)=-2sin2x , 故选:B .【点睛】本题考查了三角函数的周期、函数图象的平移,属简单题.2.B解析:B 【解析】分析】由三角函数的基本关系式和正弦的倍角公式,即可求解,得到答案. 【详解】由三角函数的基本关系式和正弦的倍角公式, 可得22sin 15cos 15sin15co 1151sin 3012454s1︒︒︒︒︒==++=++,故选B . 【点睛】本题主要考查了三角函数的基本关系式和正弦的倍角公式的应用,其中解答中熟记三角函数的基本关系式和正弦的倍角公式,准确运算是解答的关键,着重考查了运算与求解能力,属于基础题.3.B解析:B 【解析】试题分析:因为点B 是()1,2,3A 在yOz 坐标平面内的射影,所以(0,1,2)B,OB ∴==B .考点:空间中两点间的距离公式.二、填空题4.无5.【解析】 【分析】设,然后分别表示,利用正弦定理建立等式用表示,从而利用三角函数的性质得到的最小值,从而得到面积的最小值. 【详解】因为,所以, 显然,, 设,则,且, 则,所以, 在中,由正弦【解析】 【分析】设,DE x CED θ=∠=,然后分别表示,BE FEB ∠,利用正弦定理建立等式用θ表示x ,从而利用三角函数的性质得到x 的最小值,从而得到面积的最小值. 【详解】因为2010AB m AC m ==,,所以BC ==, 显然,,63B A ππ∠=∠=,设,DE x CED θ=∠=,则366EFB CEF B πππθθ∠=∠-∠=+-=+,且02πθ<<,则cos CE x θ=,所以cos BE x θ=,在BEF ∆中,由正弦定理可得:cos sin()sin66x x θππθ=+,求得x ==,其中cos 77ϕϕ==,则02πϕ<<, 因为0θϕπ<+<,所以当2πθϕ+=时,sin()θϕ+取得最大值1,则x 的最小值为7,所以面积最小值为2S ==⎝⎭【点睛】本题主要考查了利用三角函数求解实际问题的最值,涉及到正弦定理的应用,属于难题.对于这类型题,关键是能够选取恰当的参数表示需求的量,从而建立相关的函数,利用函数的性质求解最值.6.无 7.无三、解答题8.1)f ′(x )=e x +x e x -ax -a =(e x -a )(x +1). ①当a ≤0时,所以f (x )在(-∞,-1)上单调递减,在(-1,+∞上单调递增.②当a >0时,f ′(x )=0的根为x =ln a 或x =-1. 若ln a >-1,即a >1e,若ln a =-1,即a =1e, f ′(x )≥0在(-∞,+∞)上恒成立,所以f (x )在(-∞,+∞)上单调递增,无减区间.若ln a <-1,即0<a <1e,综上,当a ≤0时,f (x )在(-∞,-1)上单调递减,在(-1,+∞)上单调递增; 当0<a <1e 时,f (x )在(-∞,ln a ),(-1,+∞)上单调递增,在(ln a ,-1)上单调递减; 当a =1e时,f (x )在(-∞,+∞)上单调递增,无减区间; 当a >1e 时,f (x )在(-∞,-1),(ln a ,+∞)上单调递增,在(-1,ln a )上单调递减. (2)解法一:因为x e x -ax -a +1≥0,所以a (x +1)≤x e x +1. 当x =-1时,0≤-1e +1恒成立. 当x >-1时,a ≤x e x +1x +1.令g (x )=x e x +1x +1,g ′(x )=e x (x 2+x +1)-1(x +1)2,设h (x )=e x (x 2+x +1)-1,因为h ′(x )=e x (x +1)(x +2)>0在x ∈(-1,+∞)上恒成立, 即h (x )=e x (x 2+x +1)-1在x ∈(-1,+∞)上单调递增.又因为h (0)=0,所以g (x )=x e x +1x +1在(-1,0)上单调递减,在(0,+∞)上单调递增,则g (x )min =g (0)=1,所以a ≤1. 综上,a 的取值范围为(-∞,1].解法二:令g (x )=f (x )+a2x 2-a +1=x e x -ax -a +1, 所以g ′(x )=e x +x e x -a =e x (x +1)-a ,当a ≤0时,g ′(x )≥0,则g (x )在[-1,+∞)上单调递增, 所以g (x )≥g (-1)=-1e +1>0,满足题意. 当0<a ≤1时, 令h (x )=e x +x e x -a ,因为h ′(x )=2e x +x e x >0,即h (x )=e x +x e x -a 在[-1,+∞)上单调递增.又因为h (-1)=-a <0,h (0)=1-a ≥0,所以h (x )=e x +x e x -a =0在[-1,0]上有唯一的解,记为x 0,g (x )min =g (x 0)=x 0e 0 -ax 0-a +1=x 0e 0 -(e 0 +x 0e 0 )x 0-(e 0 +x 0e x 0 )+1=-e x 0⎣⎡⎦⎤⎝⎛⎭⎫x 0+122+34+1≥-e x 0 +1≥0,满足题意.当a >1时,g (0)=-a +1<0,不满足题意. 综上,a 的取值范围为(-∞,1].(二)选考题:10分.请考生在第22、23题中任选一题作答,如果多做,则按所做的第一题计分.9.(1)6.(2。
2020年全国普通高等学校招生统一考试(江苏卷)模拟预测卷数学试题(解析版)

2020年全国普通高等学校招生统一考试(江苏卷)模拟预测卷数学试题一、填空题1.已知集合{}1A x x =>,{}1,2,3B =,则A B =______.【答案】{}2,3【分析】根据集合交集的定义和运算,即可求解. 【详解】由题意,集合{}1A x x =>,{}1,2,3B =, 根据集合交集的定义和运算,可得{}2,3A B ⋂=. 故答案为:{}2,3.【点睛】本题主要考查了集合交集的定义及运算,其中熟记集合交集的定义是解答的关键,属于容易题.2.已知复数2z i =+(其中i 为虚数单位),若(),za bi ab R i=+∈,则ab 的值为______. 【答案】-2【分析】根据已知求出,a b ,即得解. 【详解】由题得2z ai b i =-=+, 所以2,1b a -==, 所以1a =,2b =-, 所以2ab =-. 故答案为:-2【点睛】本题主要考查复数的运算和复数相等的概念,意在考查学生对这些知识的理解掌握水平.3.已知一组数据是4,a ,7,5,8的平均数为6,则该组数据的标准差是______.【分析】首先根据平均数公式计算得到a ,再根据标准差公式计算结果. 【详解】由平均数公式475865a ++++=得6a =,所以()()()()2222146076568625s ⎡⎤=-++-+-+-=⎣⎦. 故答案为:2【点睛】本题考查样本平均数和标准差,属于基础题型.4.在平面直角坐标系xOy 中,若双曲线1C :()2210x y m m-=>的一条准线与抛物线2C :22x y =的准线重合,则正数m 的值是___.【答案】3【分析】由已知可得双曲线的准线方程及其抛物线的准线方程,即可得出正数m . 【详解】抛物线2C :22x y =的准线方程为12y,双曲线1C :221x y m-=的一条准线方程为1y m =-+,根据题意得121m =+,解得3m =. 故答案为:3【点睛】本题考查了双曲线与抛物线的标准方程及其准线方程,属于基础题. 5.运行如图的程序框图,则输出的结果是______.【答案】13【分析】根据流程图的循环结构,计算输出结果. 【详解】根据流程图可知当1i =时进入循环,12a =,当2i =时,进入循环,1121312a ==+,当3i =时退出循环,输出13a =.故答案为:13.【点睛】本题考查循环结构,重点考查理性流程图,属于基础题型.6.《易·系辞上》有“河出图,洛出书”之说,河图、洛书是中国古代流传下来的两幅神秘图案,蕴含了深奥的宇宙星象之理,被誉为“宇宙魔方”,是中华文化阴阳术数之源.河图的排列结构如图所示,一与六共宗居下,二与七为朋居上,三与八同道居左,四与九为友居右,五与十相守居中,其中白圈为阳数,黑点为阴数,若从阳数和阴数中各取一数,则其差的绝对值为5的概率为______.【答案】15【分析】根据阳数为1,3,5,7,9;阴数为2,4,6,8,10,利用古典概型的概率求法求解.【详解】∵阳数为1,3,5,7,9;阴数为2,4,6,8,10, ∴从阳数和阴数中各取一数的所有组合共有5×5=25个, 满足差的绝对值为5的有(1,6),(3,8),(5,10),(7,2),(9,4)共5个, 则其差的绝对值为5的概率为51255P == 故答案为:15【点睛】本题主要考查古典概型的概率求法,还考查了分析求解问题的能力,属于基础题.7.已知{}n a 为等差数列,n S 为其前n 项和,若2552a a +=,则15S 的值是______. 【答案】75【分析】由已知条件可解得85a =,再利用等差数列的性质即可求出.【详解】设等差数列的公差为d ,由2552a a +=,得()11524a d a d ++=+,即175a d +=,所以85a =, 则()1511581515752S a a a =+==. 故答案为:75.【点睛】本题考查等差数列性质的应用,属于基础题.8.圆柱形容器的内壁底半径是10cm ,有一个实心铁球浸没于容器的水中,若取出这个铁球,测得容器的水面下降了53cm ,则这个铁球的表面积为______2cm . 【答案】100π【分析】容器的水面下降部分的容积即为球的体积,由此计算出球的半径,再根据球的表面积公式即可求解.【详解】设实心铁球的半径为R ,则32451033R ππ=⨯⨯,得5R =, 故这个铁球的表面积为224100S R cm ππ==. 故填:100π.【点睛】本小题是立体几何的应用题,涉及圆柱的体积和球的表面积、体积的计算,考查考生理解、解决实际问题的能力. 9.若直线1y kx =+与曲线y =k 的值为______.【答案】14【分析】先求函数的导数,则0|x x k y ='==,写出切线方程与结合条件可得1,k =⎨⎪=⎪⎩,从而得出答案.【详解】y ''==,设切点为()00,x y,0y =则切线的斜率为0|x x k y ='==曲线y =()00,x y处的切线方程为y x =所以1,k =⎨⎪=⎪⎩解得14k =.故答案为:14【点睛】本题考查根据切线方程求参数的值,属于基础题. 104cos 122sin12=︒-︒______. 【答案】4-【分析】根据三角函数的基本关系式和两角和差的正弦函数公式,进行化简、运算,即可求解.【详解】原式()sin122sin 1260sin122sin 48cos12412cos 24sin122cos 24sin12cos12cos 24sin 24sin 482︒︒-︒︒︒-︒︒=====-︒︒︒︒︒︒︒︒.故答案为:4-【点睛】本题主要考查了三角函数的基本关系式,以及两角和与差的正弦公式的化简、求值,其中解答中熟记三角恒等变换的公式,准确运算是解答的关键,着重考查运算与求解能力.11.已知向量,a b ,满足3b =,a b a ⋅=,则a b -的最小值为______.【答案】【分析】利用222()2a b a b a b a b -=-=+-⋅化为数量积的运算,再代入已知条件可求得最小值.【详解】()222229218a ba b a b a a a -=+-⋅=+-=-+≥,当且仅当1a =时,等号成立,故a b -的最小值为故答案为:【点睛】本题考查求向量的模,解题关键是把向量的模转化向量数量积,然后结合函数知识得最小值.12.在平面直角坐标系xOy 中,已知A ,B 为圆C :()()2224-+-=x m y 上两个动点,且AB =若直线:2l y x =-上存在点P ,使得OC PA PB =+,则实数m 的取值范围为______.【答案】11⎡--+⎣【分析】根据题意求出AB 的中点Q 的轨迹,由2OC PA PB PQ =+=,设()00,P x y ,()11,Q x y ,进而求出点P 在以1,12D m ⎛⎫ ⎪⎝⎭为圆心,1为半径的圆D 上,根据点P 在直线l :2y x =-上,利用直线与圆的位置关系即可求解. 【详解】由题意知圆C 的圆心(),2C m ,半径2r .取AB 的中点Q ,连结CQ ,则CQ AB ⊥.所以1CQ ===, 所以点Q 在圆()()2221x m y -+-=上. 因为2OC PA PB PQ =+=,设()00,P x y ,()11,Q x y ,则()1010,PQ x x y y =--,(),2OC m =,所以()()10102,22,m x x y y ⎧=-⎪⎨=-⎪⎩则1010,21,m x x y y ⎧=+⎪⎨⎪=+⎩因为()11,Q x y 在圆()()2221x m y -+-=上, 所以()2200112m x m y ⎛⎫+-+-= ⎪⎝⎭, 即()2200112m x y ⎛⎫-+-= ⎪⎝⎭,所以点P 在以1,12D m ⎛⎫ ⎪⎝⎭为圆心,1为半径的圆D 上, 又点P 在直线l :2y x =-上,所以直线l 与圆D 有公共点,1≤,解得11m -≤-.故答案为:11⎡--⎣【点睛】本题考查了直线与圆的位置关系、轨迹问题,考查了基本知识以及知识的灵活应用,属于中档题.13.已知函数()31111,1,3442111,0,362x x x f x x x ⎧-+<≤⎪⎪=⎨⎪-+≤≤⎪⎩()()2x g x e ax a R =+-∈,若存在[]12,0,1x x ∈,使得()()12f x g x =成立,则实数a 的取值范围是______.【答案】[)2,e -+∞【分析】先利用导数求出()f x 的值域,设为集合A ,设()g x 的值域为B ,则本题等价于BA ≠∅,再求出()g x 的导数,讨论a 的范围结合()g x 的单调性和最值即可求出a 的范围.【详解】当102x ≤≤时,()f x 单调递减,()106f x ≤≤;当112x <≤时,()2104f x x '=-≥成立,()f x 单调递增,()1163f x <≤,所以()f x 的值域为10,3A ⎡⎤=⎢⎥⎣⎦. 设()g x 的值域为B ,因为存在1x ,[]20,1x ∈使得()()12f x g x =成立,所以B A ≠∅.()2x g x e ax =+-,()x g x e a '=+.①1a ≥-,任意[]0,1x ∈,()0g x '≥成立,()g x 在[]0,1单调递增, 所以()()min 01g x g ==-,()()max 12g x g e a ==+-,[]1,2B e a =-+-. 因为BA ≠∅,所以20e a +-≥,2a e ≥-;②a e ≤-,任意[]0,1x ∈,()0g x '≤成立,()g x 在[]0,1单调递减, 所以()()min 12g x g e a ==+-,()()max 01g x g ==-,[]2,1B e a =+--, 则B A ⋂=∅,不合题意; ③1e a -<<-,令()0x g x e a '=+=,()ln x a =-,()g x 在()()0,ln a -递减,()()ln ,1a -递增,所以()()()()min ln 2ln g x g a a a a =-=--+-,()()(){}max max 0,1g x g g =. 又()010g =-<,()120g e a =+-<,则B A ⋂=∅,不合题意. 综上所述,2a e ≥-.【点睛】本题考查利用导数解决能成立问题,属于较难题. 14.已知在锐角三角形ABC 中,AH BC ⊥于点H ,且()229449BA CA AH CA BA -=⋅-,若2BC =,则sin sin sin B CA的取值范围是______.【答案】5⎛⎫+∞ ⎪ ⎪⎝⎭【分析】由向量数量积的概念化简可得23BH CH =,BC 边上的高为h ,由tan ,tan B C 表示tan C ,结合三角形为锐角三角形,得h 的范围,由三角形面积公式和正弦定理结合可得2sin sin R B C h =,进而得出sin sin sin 2B C hA =,即可结果.【详解】由()229449BA CA AH CA BA -=⋅-,得229944BA AH BA CA CA AH +⋅=+⋅,所以94BA BH CA CH ⋅=⋅,即2294BH CH =,23BH CH =. 设BC 边上的高为h ,由2BC =,45BH =,6=5CH , 则5tan 4h B =,5tan 6hC =, 所以()2555046tan tan 0552524146h hh A B C h h h +=-+=-=>--⋅,所以h >因为ABC 的面积11sin 22S bc A ah ==,所以2sin sin R B C h =,所以sin sin sin 2B C h A =>.故答案为:⎫+∞⎪⎪⎝⎭.二、解答题15.在ABC 中,角A ,B ,C 所对的边分别为a ,b ,c ,且3B π=.(1)若b =2a =,求c 的值; (2)若cos A ,求cos C 的值. 【答案】(1)4c =;(2)626-.【分析】(1)根据题中所给的条件,两边一角,利用余弦定理建立等量关系式,求得c 的值;(2)根据题中所给的条件13cos A =,利用同角三角函数关系式求得23sin A =,利用诱导公式和余弦和角公式求得结果. 【详解】(1)在ABC 中,3B π=,23b =,2a =,由余弦定理得2222cos b c a ac B =+-, 得21242c c =+-,即2280c c --=, 解之得4c =或2c =-(舍去). (2)由13cos 013A =>,得02A π<<, 所以221323sin 1cos 113A A ⎛⎫=-=-= ⎪ ⎪⎝⎭. 又因为3B π=,所以()()cos cos cos C A B A B π=--=-+cos cos sin sin A B A B =-+ 1312336132-=-⨯+⨯=. 【点睛】该题考查的是有关解三角形的问题,涉及到的知识点有余弦定理解三角形,诱导公式和余弦和角公式,属于简单题目.16.已知直三棱柱111ABC A B C -,E ,F 分别是BC ,1AA 的中点,1CB CC =,AC BC ⊥.求证:(1)//EF 平面11BA C ; (2)1EF B C ⊥.【答案】(1)证明见解析;(2)证明见解析.【分析】(1)设11,B C BC 交于O 点,连接1A O ,OE ,在1BB C △中,证得1//EF A O ,结合线面平行的判定定理,即可证得//EF 平面11BA C ;(2)由直三棱柱111ABC A B C -,所以1CC ⊥平面ABC ,得到1CC AC ⊥,再由AC ⊥平面11BCC B ,得到1AC B C ⊥,证得111AC B C ⊥,进而的得到1B C ⊥平面11BA C ,即可证得1EF B C ⊥.【详解】(1)设1B C ,1BC 交于O 点,连接1A O ,OE , 在1BB C △中,点O ,E 分别是1B C ,BC 中点, 所以1//OE B B 且112OE B B =, 因为直三棱柱111ABC A B C -,所以11//B B AA ,11B B AA =,又因为F 是1AA 中点,所以1OE FA =,1//OE FA ,所以1//EF A O ,因为1AO ⊂平面11BA C ,EF ⊄平面11BA C ,所以//EF 平面11BA C . (2)因为直三棱柱111ABC A B C -,所以侧面11BCC B 是矩形, 又因为1BC CC =,所以四边形1BCC B 是正方形,所以11B C BC ⊥, 因为直三棱柱111ABC A B C -,所以1CC ⊥平面ABC , 因为AC ⊂平面ABC ,所以1CC AC ⊥, 又因为BC AC ⊥,1BCCC C =,1CC ,BC ⊂平面11BCC B ,所以AC ⊥平面11BCC B ,因为1B C ⊂平面11BCC B ,所以1AC B C ⊥,因为直三棱柱111ABC A B C -,所以11//AC A C ,所以111AC B C ⊥, 因为1111BC AC C ⋂=,1BC ,11A C ⊂平面11BAC ,所以1B C ⊥平面11BA C , 因为1AO ⊂平面11BA C ,所以11A O B C ⊥, 因为1//EF A O ,所以1EF B C ⊥.【点睛】本题主要考查了直线与平面平行的判定,以及直线与平面垂直的性质的应用,其中解答中熟记空间几何体的结构特征,以及熟练应用线面位置关系的判定定理和性质定理是解答的关键,着重考查推理与论证能力,属于中档试题.17.如图,已知边长为2的正方形材料ABCD ,截去如图所示的阴影部分后,可焊接成一个正四棱锥的封闭容器.设FCB θ∠=.(1)用θ表示此容器的体积;(2)当此容器的体积最大时,求tan θ的值. 【答案】(1))2221tan tan V θθ=-,0,4πθ⎛⎫∈ ⎪⎝⎭;(2)1tan 5θ=. 【分析】(1)取BC 的中点M ,连接FM ,连接AC 交GF 于N ,根据题意可求出正方形EFGH 的边长,进而求出底面积和高,即可求出体积; (2)令tan t θ=求出()V t 的导数,利用导数判断其单调性,从而可求出其最大值,即得解.【详解】(1)取BC 的中点M ,连接FM ,连接AC 交GF 于N ,如图.由题意知FM BC ⊥,在直角三角形CFM 中,1cos CF θ=. 在直角三角形CFN 中,sin 4NF CF πθ⎛⎫=- ⎪⎝⎭, 所以22NF θ=-,所以22GF θ=. 因为cos 4CN CF πθ⎛⎫=- ⎪⎝⎭,所以22tan CN θ=+. 从而)222GFEH S θ=,正四棱锥高2222CO CN NO CN NF =-=-222222tan tan 2tan 2222θθθ⎛⎫⎛⎫=+--= ⎪ ⎪ ⎪ ⎪⎝⎭⎝⎭所以正四棱锥的体积)211222tan 33GFEHV S CO θθ=⋅=⋅)2221tan tan θθ=-0,4πθ⎛⎫∈ ⎪⎝⎭.(2)令tan t θ=()0,1t ∈,则()))2253221233V t t t t t t =-=-+, ())()()4222222256151133V t t t t t '=-+=--. 令()0V t '=,得5t =. t50,5⎛⎫ ⎪⎝⎭555,15⎛⎫ ⎪ ⎪⎝⎭()V t '+-()V t↗ 极大值↘所以()V t 在50,⎛⎫⎪⎝⎭单调递增,在5,1⎛⎫ ⎪ ⎪⎝⎭单调递减, 所以()V t 在5t =时取到最大值,此时1tan 5θ=.【点睛】本题考查棱锥体积的求法,考查利用导数求最值,属于中档题.18.如图,点F 为椭圆C :()222210x y a b a b +=>>的左焦点,点A ,B 分别为椭圆C的右顶点和上顶点,点62,P ⎛⎫- ⎪ ⎪⎝⎭在椭圆C 上,且满足//OP AB .(1)求椭圆C 的方程; (2)过定点(),0T m ()2m <且与x 轴不重合的直线l 交椭圆C 于M ,N 两点,直线4x =分别交直线AM ,AN 于点D ,E ,求证:以DE 为直径的圆经过x 轴上的两定点(用m 表示).【答案】(1)22143x y +=;(2)证明见解析. 【分析】(1)由62,2P ⎛ ⎝⎭在椭圆上,可得222312a b +=,由//OP AB ,可得3ba=-,从而解出,a b 的值,得到答案. (2)设()11,M x y ,()22,N x y ,()00,Q x y 是以DE 为直径的圆上的任意一点,设出直线AM 的方程,得到点D 的纵坐标,同理得到点E 的纵坐标,由条件可得0DQ EQ ⋅=,得到()()()2120124422y y x x x -=---,设直线l 的方程为x ty m =+,与椭圆C 的方程22143x y +=联立,将韦达定理代入上述式子,可得答案.【详解】解:(1)由P ⎛ ⎝⎭在椭圆C :()222210x y a b a b +=>>上得222312a b +=①, 如图,由A 为C 的右顶点,B 为C 的上顶点可知(),0A a ,()0,B b , 因OPAB ,所以OP AB k k =,则b a=-②.联立①②得方程组22231,2,2a bb a ⎧+=⎪⎪⎨⎪-=-⎪⎩解得2,a b =⎧⎪⎨=⎪⎩故所求椭圆C 的方程为22143x y +=.(2)设()11,M x y ,()22,N x y ,又()2,0A , 所以直线AM 的方程为()1122y y x x =--,令4x =,得1122D yy x =-, 所以1124,2y D x ⎛⎫⎪-⎝⎭.同理2224,2y E x ⎛⎫ ⎪-⎝⎭. 设()00,Q x y 是以DE 为直径的圆上的任意一点,则0DQ EQ ⋅=,所以()21200012224022y y x y y x x ⎛⎫⎛⎫-+--= ⎪⎪--⎝⎭⎝⎭,令00y =,得()()()2120124422y y x x x -=---.设直线l 的方程为x ty m =+,与椭圆C 的方程22143x y+=联立,消去x 得()2223463120ty tmy m +++-=,所以122634tm y y t +=-+,212231234m y y t -=+, 所以()()()()12122222x x ty m ty m --=+-+-()()()()22212122422234m t y y t m y y m t -=+-++-=+.所以()()()()()()222212022122312432412334422242234m m y y m t x x x m m m t -+-+-=-=-==-----+, 因为22m -<<,所以04x =所以以DE 为直径的圆经过x 轴上两定点,其坐标分别为4⎛⎫⎪ ⎪⎝⎭和4⎛⎫⎪ ⎪⎝⎭. 【点睛】本题考查求椭圆的方程,考查直线与椭圆的位置关系,考查圆过定点问题,属于难题.19.若数列{}n c 满足:存在实数t ,使得()2212112m n m n c c c t m n --+-+=+-对任意m 、*n N ∈都成立,则称数列{}n c 为“t 倍等阶差数列”.已知数列{}n a 为“t 倍等阶差数列”.(1)若10a =,212a =-,31a =,求实数t 的值; (2)在(1)的条件下,设()*2121n n n b a a n N +-=-∈.①求数列{}n b 的通项公式;②设数列11n n b b +⎧⎫⎨⎬⎩⎭的前n 项和为n S ,是否存在正整数p 、q ,且1p q <<,使得1S 、p S 、q S 成等比数列?若存在,求出p 、q 的值,若不存在,请说明理由.【答案】(1)2t =;(2)①87n b n =-;②存在2p =,36q =.【分析】(1)由题中定义可得出关于实数t 的等式,由此可解得实数t 的值; (2)①根据题中定义可推导出数列{}n b 为等差数列,确定该数列的首项和公差,由此可求得数列{}n b 的通项公式;②利用裂项相消法可求得n S ,由题意可得出2161988p p q+=+>,可得出关于正整数p的不等式,解出p 的取值范围,可求得正整数p 的值,进而可求得q 的值,由此可得出结论.【详解】(1)由数列{}n a 为“t 倍等阶差数列”, 令2m =,1n =,得()2312221a a a t +=+-,所以11022t ⎛⎫+=⨯-+ ⎪⎝⎭,解得2t =;(2)①以2n +代替m ,得23212128n nn a a a .则()()()21212112118n n n n a a a a +-+++-⎡⎤---=⎣⎦,即18n nb b +-=. 所以数列{}n b 是以8为公差的等差数列.又1311b a a =-=,所以()18187n b n n =+-=-.②因为()()111111878188781n n b b n n n n +⎛⎫==- ⎪-+-+⎝⎭, 所以111111111189917878188181n n S n n n n ⎡⎤⎛⎫⎛⎫⎛⎫⎛⎫=-+-++-=-= ⎪ ⎪ ⎪ ⎪⎢⎥-+++⎝⎭⎝⎭⎝⎭⎝⎭⎣⎦, 则119S =,81p p S p =+,81q q S q =+. 假设1S 、p S 、q S 成等比数列,则2181981p qp q ⎛⎫=⋅⎪++⎝⎭, 因为216189988p q p q q ++==+>,所以281610p p --<, p <<又因为p 为大于1的整数,所以2p =,36q =, 所以存在2p =,36q =,使得1S 、p S 、q S 成等比数列.【点睛】本题考查数列的新定义,考查了等差数列的通项公式的求解、裂项相消法与数列的存在性问题的求解,考查计算能力,属于难题. 20.已知函数()()ln 0af x x x x=+>. (1)求函数()f x 的单调区间;(2)若函数()f x 在定义域内有两个零点,求a 的取值范围;(3)若对任意()0,x ∈+∞,不等式()()()2ln 112xm x x e x x x e++-≥-恒成立,求m的取值范围.【答案】(1)答案不唯一,见解析;(2)10,a e ⎛⎫∈ ⎪⎝⎭;(3)[)1,m ∈+∞. 【分析】(1)求导得()()20x af x x x -'=>,按0a ≤,0a >分类讨论得结果; (2)由题意得ln a x x -=在()0,∞+上有2个交点,令()ln h x x x =,则()'1ln h x x =+,得函数()h x 的单调性,最小值和最大值极限,即可得a 的取值范围;(3)由题意得()()1ln 12xm x e x e x ⎛⎫++-≥- ⎪⎝⎭,令()()1ln 21x F x m x x e e x ⎛⎫=++-+- ⎪⎝⎭,求导得()()21x m F x x e x ⎛⎫'=-+ ⎪⎝⎭,按0m ≥,0m <分类讨论得结果.【详解】(1)()()20x af x x x -'=>. 当0a ≤时,0x,得()0f x '>,所以()f x 在()0,∞+上单调增;当0a >时,令()0f x '>得x a >,所以()f x 在(),a +∞上单调增,令()0f x '<得0x a <<,所以()f x 在()0,a 上单调减.综上,当0a ≤时,()f x 的增区间为()0,∞+,无减区间; 当0a >时,()f x 的增区间为(),a +∞,减区间为()0,a . (2)令()ln 0af x x x=+=,得ln a x x -=,令()ln h x x x =,则()'1ln h x x =+, 0x,得()'0h x =的根为1=x e ,()h x ∴在10,e ⎛⎫ ⎪⎝⎭递减,在1,e ⎛⎫+∞ ⎪⎝⎭上递增,11h e e ⎛⎫∴=- ⎪⎝⎭,()0,0x h x →→,且(),x h x →+∞→+∞,要使函数()f x 有2个零点,则10a e -<-<,即10,a e ⎛⎫∈ ⎪⎝⎭;(3)0x,由()()()2ln 112xm x x e x x x e ++-≥-可得()()1ln 12x m x e x e x ⎛⎫++-≥- ⎪⎝⎭.令()()1ln 21xF x m x x e e x ⎛⎫=++-+- ⎪⎝⎭,()()()()22111x x m x m F x x e x e x x -⎛⎫'=+-=-+ ⎪⎝⎭. 当0m ≥时,20xme x+>,令()0F x '>得1x >,所以()f x 在()1,+∞上单调增; 令()0F x '<得01x <<,所以()f x 在()0,1上单调减.所以()()min 110F x F m ==-≥,得m 1≥.当0m <时,因为()()141774ln 414ln 41444F m e e m e ⎛⎫=--+-<--+- ⎪⎝⎭,即()1114ln 4044F m e ⎛⎫⎛⎫<---<⎪ ⎪⎝⎭⎝⎭,所以()0F x ≥在()0,x ∈+∞上不恒成立,则0m <舍去.综上可知,[)1,m ∈+∞.【点睛】本题主要考查了利用求导求原函数的单调性问题,同时也考查了参变分离求函数单调性与最值,进而求得参数的取值范围等,属于中档题.21.已知矩阵1002A ⎡⎤=⎢⎥⎣⎦,1201B ⎡⎤=⎢⎥⎣⎦,若直线l 依次经过变换A T ,B T 后得到直线l ':220x y +-=,求直线l 的方程.【答案】510x y +-=.【分析】本题可先设出直线l 上的任意一点(),P x y ,再设出这点经过变换T A ,T B 后得到的对应点(),P x y '''.然后根据变换对应的矩阵找到两个点的坐标的关系表达式,再根据点(),P x y '''在直线l '上,将两个点的坐标的关系表达式代入直线l '的方程即可得到直线l 的方程.【详解】解:设点(),P x y 是l 上的任意一点,其依次经过变换A T ,B T 后得到点(),P x y '''.则12100102x x y y '⎡⎤⎡⎤⎡⎤⎡⎤=⎢⎥⎢⎥⎢⎥⎢⎥'⎣⎦⎣⎦⎣⎦⎣⎦,得42x x y y y '+⎡⎤⎡⎤=⎢⎥⎢⎥'⎣⎦⎣⎦,即4,2.x x y y y ''=+⎧⎨=⎩又点P '在直线l '上,所以220x y ''+-=,故()24220x y y ++-=,即510x y +-=, 所以直线的方程为:510x y +-=【点睛】本题主要考查一条直线经过一定的变换得到对应的直线,已知其中一条直线方程求另一条直线方程,本题可通过设对应点和变换对应的矩阵找到两个点的坐标的关系表达式来求出.本题属基础题.22.已知直线l的参数方程为1222x t y m ⎧=+⎪⎪⎨⎪=+⎪⎩(t 为参数),点P (1,2)在直线l 上.(1)求m 的值;(2)以坐标原点O 为极点,x 轴的正半轴为极轴的极坐标系中,曲线C :ρ=4与直线l 交于两点A ,B 两点,求|PA |·|PB |的值. 【答案】(1)2m =;(2)11.【分析】(1)根据点P (1,2)在直线l 上,将点的坐标代入直线的参数方程求解. (2)将曲线的极坐标方程转化为直角坐标方程,然后与直线的参数方程联立,再结合韦达定理利用参数的几何意义求解. 【详解】(1)因为()1,2P ,在直线l 上,所以112,22,t m ⎧=+⎪⎪⎨⎪=⎪⎩,解得2m =+.(2)因为曲线C :ρ=4,所以曲线C 的直角坐标方程为2216x y +=,将直线l的参数方程1122x t y ⎧=+⎪⎪⎨⎪=+⎪⎩代入C的方程得(21110t t ++-=,设A ,B 所对应的参数分别为1t ,2t ,则1211t t =-, 故1211PA PB t t ==⋅.【点睛】本题主要考查极坐标方程与直角坐标方程的转化直线与圆的位置关系以及参数的几何意义的应用,还考查了运算求解的能力,属于中档题.23.设,,a b c 都是正数,求证:222()()()4()+++++≥++b c c a a b a b c a b c.【答案】见解析【分析】利用柯西不等式证明即可; 【详解】证明:因为a ,b ,c 都是正数,所以()()()()222b c c a a b a b c a b c ⎡⎤+++++++⎢⎥⎢⎥⎣⎦222222⎡⎤⎡⎤=++++⎢⎥⎢⎥⎣⎦⎢⎥⎣⎦2⎡⎤≥++⎢⎥⎣⎦()()()2b c c a a b =+++++⎡⎤⎣⎦ ()24a b c =++,所以()()()()2224b c c a a b a b c abc+++++≥++.【点睛】本题考查柯西不等式的应用,属于基础题.24.某商场准备在今年的“五一假”期间对顾客举行抽奖活动,举办方设置了,A B 两种抽奖方案,方案A 的中奖率为23,中奖可以获得2分;方案B 的中奖率为()0001P P <<,中奖可以获得3分;未中奖则不得分,每人有且只有一次抽奖机会,每次抽奖中奖与否互不影响,并凭分数兑换奖品,(1)若顾客甲选择方案A 抽奖,顾客乙选择方案B 抽奖,记他们的累计得分为X ,若3≤X 的概率为79,求0P (2)若顾客甲、顾客乙两人都选择方案A 或都选择方案B 进行抽奖,问:他们选择何种方案抽奖,累计得分的均值较大? 【答案】(1)013P =(2)当0409P <<时,他们都选择A 方案进行抽奖时,累计得分的均值较大;当0419P <<时,他们都选择B 方案进行抽奖时,累计得分的均值较大;当049P =时,他们都选择A 方案或都选择B 方案进行抽奖时,累计得分的均值相等 【分析】(1)首先求解出对立事件“5X =”的概率,再根据对立事件概率公式求得结果;(2)利用二项分布均值公式求解出()1E X 和()2E X ,根据均值的性质求得两人全选A 方案或B 方案的均值,比较两个均值的大小,得到0P 不同取值的情况下应选取的方案.【详解】(1)由已知得,甲中奖的概率为23,乙中奖的概率为0P ,且两人中奖与否互不影响记“这2人的累计得分3≤X ”的事件为C ,则事件C 的对立事件为“5X =” ()0253P X P ==()()02715139P C P X P ∴=-==-= 013P ∴= (2)设甲、乙都选择A 方案抽奖的中奖次数为1X ,都选择B 方案抽奖的中奖次数为2X 则这两人选择A 方案抽奖累计得分的均值为()12E X ,选择B 方案抽奖累计得分的均值为()23E X 由已知可得:122,3X B ⎛⎫ ⎪⎝⎭,()202,X B P()124233E X ∴=⨯=,()202E X P = ()()118223E X E X ∴==,()()220336E X E X P == 若()()1223E X E X >,则0863P > 0409P ∴<< 若()()1223E X E X <,则0863P < 0419P ∴<< 若()()1223E X E X =,则0863P = 049P ∴= 综上所述:当0409P <<时,他们都选择A 方案进行抽奖时,累计得分的均值较大 当0419P <<时,他们都选择B 方案进行抽奖时,累计得分的均值较大 当049P =时,他们都选择A 方案或都选择B 方案进行抽奖时,累计得分的均值相等 【点睛】本题考查对立事件概率的求解、二项分布均值求解及均值性质的应用问题,利用均值来解决实际问题,属于常规题型.25.已知2020220200122020(1)....x a a x a x a x -=++++(1)求122020...a a a +++的值;(2)求01220201111...a a a a ++++的值.【答案】(1)1-;(2)20211011. 【分析】(1)根据已知条件,令0x =,求得0a ,令1x =,即可求得122020...a a a +++的值;(2)由二项式定理可得()20201k k k a C=-,求得1k n C ,由120202021202112021112022k k k C C C +⎛⎫=+ ⎪⎝⎭,进而求得202001k ka =∑,即可求得答案. 【详解】(1)()20202202001220201x a a x a x a x -=+++⋅⋅⋅+——①.在①中,令0x =,得01a =.在①中,令1x =,得01220200a a a a +++⋅⋅⋅+=,∴1220201a a a ++⋅⋅⋅+=-.(2)2020220200122020(1)....x a a x a x a x -=++++由二项式定理可得()20201k k k a C =-,0k =,1,2,⋅⋅⋅,2020.()()()()()()()!!!!2!!11111!21!21!k n k n k k n k n k n k k n k n n C n n n n n --+-+++-++==⋅=⋅++++ ()()()()()111!1!1!!111121!1!2k k n n k n k k n k n n n n n n C C +++⎡⎤+-+-⎛⎫++=+=+⎢⎥ ⎪++++⎢⎥⎝⎭⎣⎦, ∴202020202000002020202011(1)(1)kk k k k k k k a C C ===-==-∑∑∑ ()20200122020202020202020202011111C C C C =-+-⋅⋅⋅+-.120202021202112021112022k k k C C C +⎛⎫=+ ⎪⎝⎭, ∴20202020011220202021020212021202120212021202112021111111(1)2022k k a C C C C C C =⎡⎤⎛⎫⎛⎫⎛⎫=+-+++-+⎢⎥ ⎪ ⎪ ⎪⎢⎥⎝⎭⎝⎭⎝⎭⎣⎦∑ 0202120212021202111202120221011C C ⎛⎫=+= ⎪⎝⎭. 【点睛】本题解题关键是掌握组合数计算方法和根据二项式定理求各项系数和步骤,考查了分析能力和计算能力,属于难题.。
2020年高考新题型填空题预测试题

2020年高考新题型填空题预测试题(1. 本期主要是训练表格式填空题,根据目前全国各省市模拟特点,表格式填空题较为流行。
2. 本期所收集和整合是全国各省市最新预测模拟题。
3. 本期内容准确地扣住高考脉搏,指明了高考填空题的动向,试题信息体现于专家提示,为考场走势导向;资源体现于题设和题解,为新纲创意示范。
Passage 1It is interesting to know what people of different nations like to do best.In France, love is the major pastime for ordinary people. When a woman enters a cafe or restaurant, she is freely discussed, not only about her appearance but about her past and future as well. She invites looks in the street whether she is beautiful or plain. There is no better way for a woman to get back her self- confidence than a walk in the streets of Paris or Marseilles.In Italy, people love talking. They sit about in cafes, exchanging news and discussing politics. They do not hesitate to ask questions about the family, incomeand private life of anybody who happens to be there.The Swiss eat as a pastime, ladies walk into a teashop, eat a couple of ice creams and a large piece of chocolate cake, and then leave, complaining about their weights.For the Americans, the greatest fondness is said to be the push of buttons. You push a button in the lift, you push a button for cigarette, chewing gums, stamps - even for a life insurance. You can even push a button and get married and another button to get divorced.In England, waiting in a line is a national passion. The English will form a line whether they have the opportunity. Long queues can be seen, for example, at stations when the train is practically empty and everybody can have a seat.根据以上内容,完成下列表格:Passage 2Dear Sunny,It's very good that you want to be friends with your neighbor, the girl who sits next to you. In my opinion, you' d better first have a heart-to-heart talk with her, because understanding each other is very important for you two. And I also think it' s better for you to ask her to join you and your classmates in all kinds of activities, such as birthday parties, going out for a walk, discussing some fashionable topics, and so on. Besides, helping each other is also very important for both of you. Just share happiness and sadness with her. And I believe she will understand you in time and it is certain you will be goodfriends in future.Good luck to you.Yours,Editor TapeHow to ask your neighbor to be your friends:Passage 3Dear editor,When I first visited the Great Wall last Sunday, I saw a foreign couple and a Chinese couple who had justfinished their lunch. Without any hesitation, the Chinese couple threw their leftover food and the plastic bags off the Wall.Astonished and shocked, the foreigners watched the waste rolling down the hill and cried out,“Why? Why here? Isn't it a bad habit to damage the environment by littering carelessly?”The astonishment and shock of our foreign friends strike my mind again and again.Shouldn't we strengthen our awareness of environment protection? The unacceptable phenomenon of spitting, littering, and picking beautiful flowers etc. in public places must: completely disappear as soon as possible.A good environment calls for active and participation by everybody.根据上文完成下列表格:Passage 4Dear Mr. Liu.I have a friend named Mark Baker. who is now teaching history in Washington University. He was born in New York in April, 1968. He began his study in Julliard Primary School in 1974 and graduated from Sidwell Friends Secondary School in 1986. Then he entered Columbia University, where he studied for more than six years and got the doctor's degree of history. Meanwhile, he studied Chinese as his second language. He has good experience in teaching and his health is in good condition.He has always dreamt of coming to China to teach Chinese students. He would be most grateful if you would give him the chance.Looking forward to hearing from you.根据上文完成下列表格:Passage 5This term our school has offered more than 10 optinal courses, from which students can choose. The courses are given from 3:30 to 5:00 every Tuesday afternoon.Students take great interest in the optional courses. Their favorites are computer, spoken English, arts and so on. They say they have learned a lot that is not taught in textbooks and they have got a great deal of practice. However, students are not quite satisfied. They hope more optional courses will be offered white their homeworkshould be less. They suggest more trips and visits should be organized so that they can learn more about what is going on outside school. Question:Passage 6A big forest fire broke out in the forest in the southwest of America at the end of November in 1992. The fire was caused by a young worker ’s smoking cigarettes. Thousands of firemen and local people tried to put out the fire, but they couldn ’t put it out in a short time . The fire lasted more than three weeks and was finally put out. In the fire nine people died and about eighty people were injured. It caused a loss at least twenty million US dollars. The fire shocked the whole country.Passage 7Dear Smith,I'm very glad that you will come to my home. For the moment, everything is well prepared here and my parents and I are looking forward to the day you come. Do phone me before you take your trip and tell me which train you're to take. I'll meet you at the station.As you know, Shanghai is a large city and there are lots of plaices worth visiting. But I think one week's time is enough. So in the first three days I' d like to show you around the city. We'll go to some business zones such as Nanjing Road. I'll take you to some famous parks as well. During the rest of the time, I plan to visit some beautiful small villages around Shanghai. You needn't bother to look for a hotel. Just stay at my home.The last thing I want to mention is that the trainticket for return should be booked in advance. So in yourphonecall before you come, tell me the date when you are going back. I'll book the ticket for you.Yours,Zhang ying***********************************************************************************************参考答案:Passage 11.Like walking in the street2.In Italy3.love ea ting4.In the USA5.like w ai ting in a linePassage 21.heart-to-heart2.Understanding each other3.to join you and your classmates in all kinds ofactivities 4.sh ar ed 5.help ea ch other Passage 3st Sunday2.my first visit to the Great Wall3.had just finished their lunch4.They threwtheir leftover food and the plastic bags off the Wall5.as tonished6.sh oc ked7.a bad habit8.damage the environment by littering carelessly9.opinion10.strengthen our awarenessPassage 41.Mark Baker2.Male4.Teacher in Washington5.B ir thplace6.Ex ce llent / in good condition7.a doctor’s degree8.Columbia 9.Chinese 10.teachPassage 51.Offered 2.Every 3.favorite 4.c om puter 5.s po ken EnglishPassage 61.in the south of America2.at the end of3.cause4.more than three weeks5.thous an ds of firemen6.lo ca l people7.deaths8.eighty9.loss 10.impact / effectPassage 71.At Zhang Ying’s home2.By train3.At the station4.Shanghai and around the city5.In thef ir st three days6.b oo ked in advance7.Everything is well prepared8.Smith 9.Make a phonecall 10.Zhang Ying。
2020高考数学填空题型精选精练(28)

2020高考数学填空题型精选精练1、已知实数x 、y 满足方程()()22111x a y -++-=,当0y b ≤≤(b R ∈)时,由此方程可以确定一个偶函数()y f x =,则抛物线212y x =-的焦点F 到点(,)a b 的轨迹上点的距离最大值为________.2、已知βα,为锐角,且6πβα=-,那么βαsin sin 的取值范围是 . 3、有下列四个命题:(1)一定存在直线l ,使函数1()lg lg2f x x =+的图像与函数2)lg()(+-=x xg 的图像关于直线l 对称;(2)在复数范围内,00,0a bi a b +=⇔==(3)已知数列{}n a 的前n 项和为1(1)n n S =--,n N *∈,则数列{}n a 一定是等比数列; (4)过抛物线22(0)y px p =>上的任意一点(,)M x y o o 的切线方程一定可以表示为 00()y y p x x =+.则正确命题的序号为__________.4、有n 个小球,将它们任意分成两堆,求出这两堆小球球数的乘积,再将其中一堆小球任意分成两堆,求出这两堆小球球数的乘积,如此下去,每次都任选一堆,将这堆小球任意分成两堆,求出这两堆小球球数的乘积,直到不能再分为止,则所有乘积的和为 .5、设奇函数()f x 在(0)+∞,上为增函数,且(1)0f =,则不等式()()0f x f x x--<的解集为 . 6、已知抛物线)0(22>=p px y 焦点F 恰好是双曲线22221x y a b -=的右焦点,且双曲线过点(2232,a b p p),则该双曲线的渐近线方程为__________.7、已知函数22log (1),0,()2,0.x x f x x x x +>⎧=⎨--≤⎩ 若函数()()g x f x m =-有3个零点,则实数m 的取值范围是__________.8、当210≤≤x 时,21|2|3≤-x ax 恒成立,则实数a 的取值范围是__________.9、首项为正数的数列{}n a 满足211(3),.4n n a a n N ++=+∈ ,若对一切n N +∈都有1n n a a +>,则1a 的取值范围是__________.10、已知点O 为ABC ∆24==,则=• .11、在一个密封的容积为1的透明正方体容器内装有部分液体,如果任意转动该正方体,液面的形状都不可能是三角形,那么液体体积的取值范围是 .12、对于函数)(x f ,在使)(x f ≥M 恒成立的所有常数M 中,我们把M 中的最大值称为函数)(x f 的“下确界”,则函数22)1(1)(++=x x x f 的下确界为 .13、三位同学合作学习,对问题“已知不等式222xy ax y ≤+对于[][]1,2,2,3x y ∈∈恒成立,求a 的取值范围”提出了各自的解题思路.甲说:“可视x 为变量,y 为常量来分析”.乙说:“不等式两边同除以x 2,再作分析”.丙说:“把字母a 单独放在一边,再作分析”.参考上述思路,或自已的其它解法,可求出实数a 的取值范围是 .14、已知||2||0a b =≠r r ,且关于x 的函数3211()||32f x x a x a bx =++⋅r r r 在R 上有极值,则a r 与b r 的夹角范围为__________.参考答案1;2、⎥⎥⎦⎤ ⎝⎛+423,0;3、(3)(4);4、()12n n -;5、 )1,0()0,1(⋃-;6、y x = 7、(0,1);8、1322a -≤≤;9、101a <<或13a >;10、6;11、)65,61(;12、0.5 13、),1[+∞-;14、]3ππ,(。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
Passage 2
Dear Sunny,
It's very good that you want to be friends with your
neighbor, the girl who sits next to you. In my opinion,
you' d better first have a heart-to-heart talk
the Wall.
Astonished
and shocked, the foreigners watched the
waste rolling down the hill and cried out, “ Why?Whyhere?
Isn't it a bad habit to damage the environment by
Editor
Tape
How to ask your neighbor to be your friends:
What you should The reason why you
do.
should do so.
1. Have a 1 talk 1. 2 is important for
with her.
In England, waiting in a line is a national passion.
The English will form a line whether they have the
opportunity. Long queues can be seen, for example, at
the two of you.
2. It' s better to ask
2. Good friends should have
her 3 .
4 activities.
3.Learn to 5 .
3. Because it's important
for both of you.
Passage 3 Dear editor, When I first visited the Great Wall last Sunday,
stations when the train is practically
empty and
everybody can have a seat.
根据以上内容,完成下列表格:
In different countries
What they like to do
best
In
France,
a
Frenchwoman get back 1.___________________
you push a button for cigarette, chewing gums, stamps -
even for a life insurance. You can even push a button and
get married and another button to get divorced.
than six years and got the doctor's degree of history.
Meanwhile, he studied Chinese as his second language. He
has good experience in teaching and his health is in good
Students take great interest in the optional courses. Their favorites are computer, spoken English, arts and so on. They say they have learned a lot that is not taught in textbooks and they have got a great deal of practice. However, students are not quite satisfied. They hope more optional courses will be offered white their homework
chocolate cake, and then leave, complaining about their
weights.
For the Americans, the greatest fondness is said
to be the push of buttons. You push a button in the lift,
In Italy, people love talking. They sit about in cafes, exchanging news and discussing politics. They do not hesitate to ask questions about the family, income
A good environment calls for active and participation by
everybody.
根据上文完成下列表格 :
Time:
1 . _________________________
activity
2.__________________________
What. saw
protection.
Passage 4
Dear Mr. Liu.
I
have a friend named Mark Baker. who is now
teaching history in Washington University. He was born
in New York in April,
and private life of anybody who happens to be there.
The Swiss eat as a pastime, ladies walk into a
teashop, eat a couple of ice creams and a large piece of
her
self- confidence
2.____________________ Like talking
In Switzerland
3.____________________
4.____________________ Like pushing buttons
In England
5.____________________
with her,
because understanding each other is very important for
you two. And I also think it' s better for you to ask her
to join you and your classmates in all kinds of activities,
happiness and sadness with her. And I believe she will
understand you in time and it is certain you will be good
friends in future. Good luck to you.
Yours,
根据上文完成下列表格 :
Name: (1) ___ Sex: (2) ____ Nationality
Work: (4) ___
______
_____
(3) _____
______
(5)_ _____:N
Date of birth: April, Health: (6) _
ew York
1968
________
Degree: ___
_(7)___ of
Second
His dream: Coming to
History at language: (9)
China to
(8)
(10)
.
University
Passage 5
This term our school has offered more than 10 optinal courses, from which students can choose. The courses are given from 3:30 to 5:00 every Tuesday afternoon.
It is interesting to know what people of different nations like to do best.
In France, love is the major pastime for ordinary people. When a woman enters a cafe or restaurant, she is freely discussed, not only about her appearance but about her past and future as well. She invites looks in the street whether she is beautiful or plain. There is no better way for a woman to get back her self- confidence than a walk in the streets of Paris or Marseilles.
I saw a foreign couple and a Chinese couple who had just
