《固定收益证券》课程第六次作业
固定收益证券作业

固定收益证券作业固定收益证券作业11. The amount an investor will have in 15 years if $1,000 is invested today at an annual interest rate of 9% will be closest to:A. $1,350.B. $3,518.C. $3,642.2. F ifty years ago, an investor bought a share of stock for $10. T he stock has paid no dividends during this period, yet it has returned 20%, compounded annually, over the past 50 years.I f this is true, the share price is now closest to:A. $4,550.B. $45,502.C. $91,004.3. How much must be invested today at 0% to have $100 in three years?A. $77.75.B. $100.00.C. $126.30.4. H ow much must be invested today, at 8% interest, to accumulate enough to retire a $10,000 debt due seven years from today? T he amount that must be invested today is closest to:A. $5,835.B. $6,123.C. $8,794.5. An analyst estimates that XYZ’s earnings will grow from $3.00 a share to $4.50 per share over the next eight years. T he rate of growth in XYZ’s earnings is closest to:A. 4.9%.B. 5.2%.C. 6.7%.6. I f $5,000 is invested in a fund offering a rate of return of 12% per year, approximately how many years will it take for the investment to reach $10,000?A. 4 years.B. 5 years.C. 6 years.7. An investment is expected to produce the cash flows of $500, $200, and $800 at the end of the next three years. I f the required rate of return is 12%, the present value of this investment is closest to:A. $835.B. $1,175.C. $1,235.8. Given an 8.5% discount rate, an asset that generates cash flows of $10 in year 1, –$20 in year 2, $10 in year 3, and is then sold for $150 at the end of year 4, has a present value of:A. $108.29.B. $135.58.C. $163.42.9. An investor has just won the lottery and will receive $50,000 per year at the end of each of the next 20 years. A t a 10% interest rate, the present value of the winnings is closest to:A. $425,678.B. $637,241.C. $2,863,750.10. If $10,000 is invested today in an account that earns interest at a rate of 9.5%, what is the value of the equalwithdrawals that can be taken out of the account at the end of each of the next five years if the investor plans to deplete the account at the end of the time period?A. $2,453.B. $2,604.C. $2,750.11. A n investor is to receive a 15-year $8,000 annuity, the first payment to be received today.A t an 11% discount rate, this annuity’s worth today is closest to:A. $55,855.B. $57,527.C. $63,855.12. Given an 11% rate of return, the amount that must be put into an investment account at the end of each of the next ten years in order to accumulate $60,000 to pay for a child’s education is closest to:A. $2,500.B. $3,588.C. $4,432.13. A n investor will receive an annuity of $4,000 a year for ten years. T he first payment is to be received five years from today. A t a 9% discount rate, this annuity’s worth today is closest to:A. $16,684.B. $18,186.C. $25,671.14. If $1,000 is invested today and $1,000 is invested at the beginning of each of the next three years at 12% interest (compounded annually), the amount an investor will have at theend of the fourth year will be closest to:A. $4,779.B. $5,353.C. $6,792.15. A n investor is looking at a $150,000 home. I f 20% must be put down and the balance is financed at 9% over the next 30 years, what is the monthly mortgage payment?A. $799.33.B. $895.21.C. $965.55.16. Given daily compounding, the growth of $5,000 invested for one year at 12% interest will be closest to:A. $5,600.B. $5,628.C. $5,637.17. T erry C orporation preferred stocks are expected to paya $9 annual dividend forever. I f the required rate of return on equivalent investments is 11%, a share of Terry preferred should be worth:A. $81.82.B. $99.00.C. $122.22.18. A share of George C o. preferred stock is selling for $65.I t pays a dividend of $4.50 per year and has a perpetual life. T he rate of return it is offering its investors is closest to:A. 4.5%.B. 6.9%.C. 14.4%.19. If $10,000 is borrowed at 10% interest to be paid back over ten years, how much of the second year’s payment isinterest (assume annual loan payments)?A. $937.26.B. $954.25.C. $1,037.26.20. What is the effective annual rate for a credit card that charges 18% compounded monthly?A. 15.38%.B. 18.81%.C. 19.56%.分析题1. T he Parks plan to take three cruises, one each year. T hey will take their first cruise 9 years from today, the second cruise one year after that, and the third cruise 11 years from today.T he type of cruise they will take currently costs $5,000, but they expect inflation will increase this cost by 3.5% per year on average. T hey will contribute to an account to save for these cruises that will earn 8% per year. What equal contributions must they make today and every year until their first cruise (ten contributions) in order to have saved enough for all three cruises at that time? T hey pay for cruises when taken.2. A company’s dividend in 1995 was $0.88. O ver the next eight years, the dividends were $0.91, $0.99, $1.12, $0.95, $1.09, $1.25, $1.42, $1.26. Calculate the annually compounded growth rate of the dividend over the whole period.3. An investment (a bond) will pay $1,500 at the end of each year for 25 years and on the date of the last payment will also make a separate payment of $40,000. If your required rate of return on this investment is 4%, how much would you be willing to pay for the bond today?4. A bank quotes certificate of deposit (CD) yields both asannual percentage rates (A P R) without compounding and as annual percentage yields (A PY) that include the effects of monthly compounding. A$100,000 CD will pay $110,471.31 at the end of the year. C alculate the A P R and A PY the bank is quoting.5. Aclient has $202,971.39 in an account that earns 8% per year, compounded monthly. T he client’s 35th bir thday was yesterday and she will retire when the account value is $1 million.A. At what age can she retire if she puts no more money in the account?B. At what age can she retire if she puts $250/month into the account every month beginningone month from today?6. At retirement nine years from now, a client will have the option of receiving a lump sum of £400,000 or 20 annual payments of £40,000 with the first payment made at retirement. What is the annual rate the client would need to earn on a retirement investment to be indifferent between the two choices?Use the following data to answer Questions 1 and 1Assume an investor purchases a share of stock for $50 at time t = 0, and another share at $65 at time t = 1, and at the end of year 1 and year 2, the stock paid a $2.00 dividend. Also, at the end of year 2, the investor sold both shares for $70 each.1. The money-weighted rate of return on the investment is:A. 15.45%.B. 16.73%.C. 18.02%.2. The time-weighted rate of return on the investment is:A. 18.27%.B. 20.13%.C. 21.83%.3 What is the bank discount yield for a T-bill that is selling for $99,000, with a face value of $100,000, and 95 days remaining until maturity?A. 1.51%.B. 3.79%.C. 6.00%.4. What is the holding period yield for a T-bill that is selling for $99,000 if it has a face value of $100,000 and 95 days remaining until maturity?A. 1.01%.B. 2.03%.C. 3.79%.5. What is the effective annual yield for a T-bill that is selling for $99,000 if it has a face value of $100,000 and 95 days remaining until maturity?A. 3.79%.B. 3.94%.C. 4.50%.6. What is the money market yield for a T-bill that is selling for $99,000 if it has a face value of $100,000 and 95 days remaining until maturity?A. 3.79%.B. 3.83%.C. 3.90%.7. Which of the following is least accurate regarding a bank discount yield?A. It ignores the opportunity to earn compound interest.B. It is based on the face value of the bond, not its purchase price.C. It reflects the nonannualized return an investor will earnover a sec urity’s life.8. A 175-day T-bill has an effective annual yield of 3.80%. I ts money-market yield is closest to:A. 1.80%.B. 3.65%.C. 3.71%.1. A A llison R ogers, CFACFA, makes the following statement: “T he problems with bank discount yields quoted for T-bills is that they aren’t yields, they ignore compounding, and they are based on a short year.”A. I I s she correct in all regards?B. Which of these problems is/are remedied by using the holding period yield?C. Which of these problems is/are remedied by using a money market yield?D. Which of these problems is/are remedied by using effective annual yields?2. L. A dams buys 1,000 shares of Morris T ool stock for $35 per share. O ne year later the stock is $38 per share and has paid a dividend of $1.50 per share. A dams reinvests the dividends in additional shares at the time. A t the end of the second year, the shares are trading for $37 and have paid $2 dividends over the period.L. Burns buys 500 shares of Morris T ool stock for $35 per share. O ne year later the stock is $38 per share and has paid a dividend of $1.50 per share. Burns reinvests the dividends in additional shares at that time and also buys 500 additional shares.A t the end of the second year, the shares are trading for $37 and have paid $2 in dividends over the period.A. C C ompare the annual time-weighted returns for theaccounts of the two investors (no calculation required).B. C C ompare the annual money-weighted returns for the accounts of the two investors (no calculation required).。
固定收益证券作业及答案

作业一1.三年后收到的$100,现在的价值是多少?假设三年期零息债券的利率是:a.复利20%,年计息b.复利100%,年计息c.复利0%,年计息d.复利20%,半年计息e.复利20%,季计息f.复利20%,连续计息2.以连续复利方式计息,下列利率各为多少?a.复利4%,年计息b.复利20%,年计息c.复利20%,季计息d.复利100%,年计息3.考虑下列问题:a.华尔街日报在交易日92年9月16日给出了票面利率为9 1/8’s在92年12月31日到期,92年9月17日结算的政府债券,其标价为买入价101:23,卖出价101:25。
这种债券相应买入和卖出的收益率是多少?b.在同一交易日,华尔街日报对同时在92年12月31日到期和在92年9月17日结算的T-bill报出的买入和卖出折现率分别是2.88%和2.86%(附录A“利率报价和惯例”中的例14中提到的T-bill),这里面是不是有套利机会?(“买入”和“卖出”是从交易者的角度出发,你是以“买入价”卖出,以“卖出价”买入)。
4.你在交易日92年9月16日以$10-26买入$2,000万票面价值为100的在2021年11月15日到期的STRIPs(零息债券),这种债券的到期收益率是多少?5.今天是交易日,1994年10月10日,星期一。
这三种债券的面值均为$100,每半年付息一次。
注意到上表中最后一列是到期收益,它反映了给定到期日,某种特定债券的标准惯例。
在计算日期时,不考虑闰年,同时也要忽略假期。
回答这个问题时非常重要的是要写清楚你的运算过程。
不能只是用计算器把价格计算出来。
本题要想得分,必须把计算中的所有步骤都写清楚。
a.计算美国财政部发行的国债的报价,假定此国债是按标准结算方式结算。
b.计算费城发行的城市债券的报价,假定此类债券标准结算期为三天。
c.计算联邦全国抵押协会发行的机构债券的报价,假定此类债券是按标准结算方式结算。
作业二1.考虑一固定付息债券,每年支付利息$1009.09,利息于时期1,2,…20支付。
固定收益证券作业

固定收益证券作业 11.Theamountaninvestorwillhavein15yearsif$1,000isinvestedtodayatanannualinterestrateof9%willbe closes t to:A.$1,350.B.$3,518.C.$3,642.2.F iftyyearsago,aninvestorboughtashareofstockfor$10.T hestockhaspaidnodividendsduringthisperiod,yetit hasreturned20%,compoundedannually,overthepast50years.I fthisistrue,thesharepriceisnow closest to:A.$4,550.B.$45,502.C.$91,004.3.Howmuchmustbeinvestedtodayat0%tohave$100inthreeyearsA.$77.75.B.$100.00.C.$126.30.4.H owmuchmustbeinvestedtoday,at8%interest,toaccumulateenoughtoretirea$10,000debtduesevenyearsfro mtoday T heamountthatmustbeinvestedtodayis closest to:A.$5,835.B.$6,123.C.$8,794.5.AnanalystestimatesthatXYZ’searningswillgrowfrom$3.00ashareto$4.50pershar eoverthenexteightyears.T herateofgrowthinXYZ’searningsis closest to:6.I f$5,000isinvestedinafundofferingarateofreturnof12%peryear,approximatelyhowmanyyearswillittakefor theinvestmenttoreach$10,000A.4years.B.5years.C.6years.7.Aninvestmentisexpectedtoproducethecashflowsof$500,$200,and$800attheendofthenextthreeyears.I fther equiredrateofreturnis12%,thepresentvalueofthisinvestmentis closest to:A.$835.B.$1,175.C.$1,235.A.$108.29.B.$135.58.C.$163.42.9.Aninvestorhasjustwonthelotteryandwillreceive$50,000peryearattheendofeachofthenext20years.A ta10%i nterestrate,thepresentvalueofthewinningsis closest to:A.$425,678.B.$637,241.C.$2,863,750.10.If$10,000isinvestedtodayinanaccountthatearnsinterestatarateof9.5%,whatisthevalueoftheequalwithdra walsthatcanbetakenoutoftheaccountattheendofeachofthenextfiveyearsiftheinvestorplanstodepletetheaccou ntattheendofthetimeperiodA.$2,453.B.$2,604.C.$2,750.11.A ninvestoristoreceivea15-year$8,000annuity,thefirstpaymenttobereceivedtoday.A tan11%discountrate, thisannuity’sworthtodayis closest to:A.$55,855.B.$57,527.C.$63,855.12.Givenan11%rateofreturn,theamountthatmustbeputintoaninvestmentaccountattheendofeachofthenextten yearsinordertoaccumulate$60,000topayforachild’seducationis closest to:A.$2,500.B.$3,588.C.$4,432.13.A ninvestorwillreceiveanannuityof$4,000ayearfortenyears.T hefirstpaymentistobereceivedfiveyearsfro mtoday.A ta9%discountrate,thisannuity’sworthtodayis closest to:A.$16,684.B.$18,186.C.$25,671.14.If$1,000isinvestedtodayand$1,000isinvestedatthebeginningofeachofthenextthreeyearsat12%interest(co mpoundedannually),theamountaninvestorwillhaveattheendofthefourthyearwillbe closest to:A.$4,779.B.$5,353.C.$6,792.15.A ninvestorislookingata$150,000home.I f20%mustbeputdownandthebalanceisfinancedat9%overthenext 30years,whatisthemonthlymortgagepaymentA.$799.33.B.$895.21.C.$965.55.16.Givendailycompounding,thegrowthof$5,000investedforoneyearat12%interestwillbe closest to:A.$5,600.B.$5,628.C.$5,637.17.T erry C orporationpreferredstocksareexpectedtopaya$9annualdividendforever.I ftherequiredrateofreturn onequivalentinvestmentsis11%,ashareofTerrypreferredshouldbeworth:A.$81.82.B.$99.00.C.$122.22.18.A shareofGeorge C o.preferredstockissellingfor$65.I tpaysadividendof$4.50peryearandhasaperpetuallife. T herateofreturnitisofferingitsinvestorsis closest to:19.If$10,000isborrowedat10%interesttobepaidbackovertenyears,howmuchofthesecondyear’spaymentisint erest(assumeannualloanpayments)A.$937.26.B.$954.25.C.$1,037.26.20.Whatistheeffectiveannualrateforacreditcardthatcharges18%compoundedmonthly分析题1.T heParksplantotakethreecruises,oneeachyear.T heywilltaketheirfirstcruise9yearsfromtoday,thesecondcru iseoneyearafterthat,andthethirdcruise11yearsfromtoday.T hetypeofcruisetheywilltakecurrentlycosts$5,000, buttheyexpectinflationwillincreasethiscostby3.5%peryearonaverage.T heywillcontributetoanaccounttosave forthesecruisesthatwillearn8%peryear.Whatequalcontributionsmusttheymaketodayandeveryyearuntiltheirf irstcruise(tencontributions)inordertohavesavedenoughforallthreecruisesatthattime T heypayforcruiseswhent aken.2.A company’sdividendin1995was$0.88.O3.Aninvestment(abond)willpay$1,500attheendofeachyearfor25yearsandonthedateofthelastpaymentwillals omakeaseparatepaymentof$40,000.Ifyourrequiredrateofreturnonthisinvestmentis4%,howmuchwouldyoub ewillingtopayforthebondtoday4.A bankquotescertificateofdeposit(CD)yieldsbothasannualpercentagerates(A P R)withoutcompoundingand asannualpercentageyields(A PY)thatincludetheeffectsofmonthlycompounding.A$100,000CD willpay$110, 471.31attheendoftheyear.C alculatethe A P R and A PYthebankisquoting.5.Aclienthas$202,971.39inanaccountthatearns8%peryear,compoundedmonthly.T heclient’s35thbirthdayw asyesterdayandshewillretirewhentheaccountvalueis$1million.A.AtwhatagecansheretireifsheputsnomoremoneyintheaccountB.Atwhatagecansheretireifsheputs$250/monthintotheaccounteverymonthbeginningonemonthfromtoday6.Atretirementnineyearsfromnow,aclientwillhavetheoptionofreceivingalumpsumof£400,000or20annualpaymentsof£40,000withthefirstpaymentmadeatretirement.Whatistheannualratetheclientwouldneedtoearnonaretireme ntinvestmenttobeindifferentbetweenthetwochoicesUsethefollowingdatatoanswerQuestions1and11.Themoney-weightedrateofreturnontheinvestmentis:2.Thetime-weightedrateofreturnontheinvestmentis:3Whatisthebankdiscountyieldfora T-billthatissellingfor$99,000,withafacevalueof$100,000,and95daysrema ininguntilmaturity4.Whatistheholdingperiodyieldfora T-billthatissellingfor$99,000ifithasafacevalueof$100,000and95daysre maininguntilmaturity5.Whatistheeffectiveannualyieldfora T-billthatissellingfor$99,000ifithasafacevalueof$100,000and95daysre maininguntilmaturity6.Whatisthemoneymarketyieldfora T-billthatissellingfor$99,000ifithasafacevalueof$100,000and95daysre maininguntilmaturity7.Whichofthefollowingis leastaccurate regardingabankdiscountyieldA.Itignorestheopportunitytoearncompoundinterest.B.Itisbasedonthefacevalueofthebond,notitspurchaseprice.C.Itreflectsthenonannualizedreturnaninvestorwillearnoverasecurity’slife.8.A175-day T-billhasaneffectiveannualyieldof3.80%.I tsmoney-marketyieldis closest to:A.1.80%.C.3.71%.1.AA llison R ogers,CFACFA,makesthefollowingstatement:“T heproblemswithbankdiscountyieldsquotedfor T -billsisthattheyaren’tyields,theyignorecompounding,andtheyarebasedonashortyear.”A.II sshecorrectinallregardsB.Whichoftheseproblemsis/areremediedbyusingtheholdingperiodyieldC.Whichoftheseproblemsis/areremediedbyusingamoneymarketyieldD.Whichoftheseproblemsis/areremediedbyusingeffectiveannualyields2.L.A damsbuys1,000sharesofMorris T oolstockfor$35pershare.O neyearlaterthestockis$38pershareandhaspaidadividendof$1.50pershare.A damsreinveststhedividendsinadditionalsharesatthetime.A ttheendofthesecon dyear,thesharesaretradingfor$37andhavepaid$2dividendsovertheperiod.L.Burnsbuys500sharesofMorris T oolstockfor$35pershare.OA ttheendofthesecondyear,thesharesaretradingf or$37andhavepaid$2individendsovertheperiod. omparetheannualtime-weightedreturnsfortheaccountsofthetwoinvestors(nocalculationrequired). omparetheannualmoney-weightedreturnsfortheaccountsofthetwoinvestors(nocalculationrequired).。
对外经济贸易大学国际经济贸易学院《固定收益证券》部分答案

国际经济贸易学院研究生课程班《固定收益证券》试题1)Explain why you agree or disagree with the following statement: “The price of a floater will always trade at its par value.”Answer:I disagree with the statement: “The price of a floater will always trade at its par value.” F irst, the coupon rate of a floating-rate security (or floater) is equal to a reference rate plus some spread or margin. For example, the coupon rate of a floater can reset at the rate on a three-month Treasury bill (the reference rate) plus 50 basis points (the spread). Next, the price of a floater depends on two factors: (1) the spread over the reference rate and (2) any restrictions that may be imposed on the resetting of the coupon rate. For example, a floater may have a maximum coupon rate called a cap or a minimum coupon rate called a floor. The price of a floater will trade close to its par value as long as (1) the spread above the reference rate that the market requires is unchanged and (2) neither the cap nor the floor is reached. However, if the market requires a larger (smaller) spread, the price of a floater will trade below (above) par. If the coupon rate is restricted from changing to the reference rate plus the spread because of the cap, then the price of a floater will trade below par.2) A portfolio manager is considering buying two bonds. Bond A matures in three years and has a coupon rate of 10% payable semiannually. Bond B, of the same credit quality, matures in 10 years and has a coupon rate of 12% payable semiannually. Both bonds are priced at par.(a) Suppose that the portfolio manager plans to hold the bond that is purchased for three years. Which would be the best bond for the portfolio manager to purchase? Answer:The shorter term bond will pay a lower coupon rate but it will likely cost less for a given market the bonds are of equal risk in terms of creit quality (The maturity premium for the longer term bond should be greater),the question when comparing the two bond investments is:What investment will be expecte to give the highest cash flow per dollar invested?In other words,which investment will be expected to give the highest effective annual rate of general,holding the longer term bond should compensate the investor in the form of a maturity premium and a higher expected ,as seen in the discussion below,the actual realized return for either investment is not known with certainty.To begin with,an investor who purchases a bond can expect to receive a dollar return from(i)the periodic coupon interest payments made be the issuer,(ii)an capital gain when the bond matures,is called,or is sold;and (iii)interest income generated from reinvestment of the periodic cash last component of the potential dollar return is referred to as reinvestment a standard bond(our situation)that makes only coupon payments and no periodic principal payments prior to the maturity date,the interim cash flows are simply the coupon ,for such bonds the reinvestment income is simply interest earned from reinvesting the coupon interest these bonds,the third component of the potential source of dollar return is referred to as theinterest-on-interest components.If we are going to coupute a potential yield to make a decision,we should be aware of the fact that any measure of a bond’s potential yield should take into consideration each of the three components described current yield considers only the coupon interest consideration is given to any capital gain or interest on yield to maturity takes into account coupon interest and any capital also considers the interest-on-interest ,implicit in the yield-to-maturity computation is the assumption that the coupon payments can be reinvested at the computed yield to yield to maturity is a promised yield and will be realized only if the bond is held to maturity and the coupon interest payments are reinvested at the yield to the bond is not held to maturity and the coupon payments are reinvested at the yield to maturity,then the actual yield realized by an investor can be greater than or less than the yield to maturity.Given the facts that(i)one bond,if bought,will not be held to maturity,and(ii)the coupon interest payments will be reinvested at an unknown rate,we cannot determine which bond might give the highest actual realized ,we cannot compare them based upon this ,if the portfolio manager is risk inverse in the sense that she or he doesn’t want to buy a longer term bond,which will likel have more variability in its return,then the manager might prefer the shorter term bond(bondA) of thres bond also matures when the manager wants to cash in the ,the manager would not have to worry about any potential capital loss in selling the longer term bond(bondB).The manager would know with certainty what the cash flowsThese cash flows are spent when received,the manager would know exactly how much money could be spent at certain points in time.Finally,a manager can try to project the total return performance of a bond on the basis of the panned investment horizon and expectations concerning reinvestment rates and future market ermits the portfolio manager to evaluate thich of several potential bonds considered for acquisition will perform best over the planned investment we just rgued,this cannot be done using the yield to maturity as a measure of relative total return to assess performance over some investment horizon is called horizon a total return is calculated oven an investment horizon,it is referred to as a horizon horizon analysis framwor enabled the portfolio manager to analyze the performance of a bond under different interest-rate scenarios for reinvestment rates and future market by investigating multiple scenarios can the portfolio manager see how sensitive the bond’s performance will be to each can help the manager choose between the two bond choices.(b) Suppose that the portfolio manager plans to hold the bond that is purchased for six years instead of three years. In this case, which would be the best bond for the portfolio manager to purchase?Answer:Similear to our discussion in part(a),we do not know which investment would give the highest actual relized return in six years when we consider reinvesting all cash the manager buys a three-year bond,then there would be the additional uncertainty of now knowing what three-year bond rates would be in three purchase of the ten-year bond would be held longer than previously(six years compared to three years)and render coupon payments for a six-year period that are these cash flows are spent when received,the manager will know exactly how much money could be spentat certain points in timeNot knowing which bond investment would give the highest realized return,the portfolio manager would choose the bond that fits the firm’s goals in terms of maturity.3) Answer the below questions for bonds A and B.Bond A Bond BCoupon8%9%Yield to maturity8%8%Maturity (years)25Par$$Price$$(a) Calculate the actual price of the bonds for a 100-basis-point increase in interest rates.Answer:For Bond A, we get a bond quote of $100 for our initial price if we have an 8% coupon rate and an 8% yield. If we change the yield 100 basis point so the yieldis 9%, then the value of the bond (P) is the present value of the coupon payments plus the present value of the par value. We have C = $40, y = %, n = 4, and M = $1,000. Inserting these numbers into our present value of coupon bond formula, we get:The present value of the par or maturity value of $1,000 is:Thus, the value of bond A with a yield of 9%, a coupon rate of 8%, and a maturity of 2 years is: P = $ + $ = $. Thus, we get a bond quote of $. We already know that bond B will give a bond value of $1,000 and a bond quote of $100 since a change of 100 basis points will make the yield and coupon rate the same, For example, inserting Thus, the value of bond A with a yield of 9%, a coupon rate of 8%, and a maturity of 2 years is: P = $ + $ = $. Thus, we get a bond quote of $. We already know that bond B will give a bond value of $1,000 and a bond quote of $100 since a change of 100 basis points will make the yield and coupon rate the same, For example, inserting (b) Using duration, estimate the price of the bonds for a 100-basis-point increase in interest rates.Answer:To estimate the price of bond A, we begin by first computing the modified duration. We can use an alternative formula that does not require the extensive calculations required by the Macaulay procedure. The formula is:Putting all applicable variables in terms of $100, we have C = $4, n = 4, y = , and P = $. Inserting these values, in the modified duration formula gives:($1,[] + $ / $ = ($ + $ / $ = $ / $ = or about . Converting to annual number by dividing by two gives a modified duration of (before the increase in 100 basis points it was . We next solve for the change in price using the modified duration of and dy = 100 basis points = . We have:We can now solve for the new price of bond A as shown below:This is slightly less than the actual price of $. The difference is $ – $ = $. To estimate the price of bond B, we follow the same procedure just shown for bond A. Using the alternative formula for modified duration that does not require the extensive calculations required by the Macaulay procedure and noting that C = $45, n = 10, y = , and P = $100, we get:($ + $0) / $100 = or about (before the increase in 100 basis points it was or about . Converting to an annual number by dividing by two gives a modified duration of (before the increase in 100 basis points it was . We will now estimate the price ofbond B using the modified duration measure. With 100 basis points giving dy = and an approximate duration of , we have:Thus, the new price is(1 – $1, = $1, = $.This is slightly less than the actual price of $1,000. The difference is $1,000 – $ = $.(c) Using both duration and convexity measures, estimate the price of the bonds for a 100-basis-point increase in interest rates.Answer:For bond A, we use the duration and convexity measures as given below. First, we use the duration measure. We add 100 basis points and get a yield of 9%. We now have C = $40, y = %, n = 4, and M = $1,000. NOTE. In part (a) we computed the actual bond price and got P = $. Prior to that, the price sold at par (P = $1,000) since the coupon rate and yield were then equal. The actual change in price is: ($ – $1,000) = ?$ and the actual percentage change in price is: ?$ / $1,000 = ?%. We will now estimate the price by first approximating the dollar price change. With 100 basis points giving dy = and a modified duration computed in part (b) of , we have: This is slightly more negative than the actual percentage decrease in price of ?%. The difference is ?% – (?%) = ?% + % = %. Using the ?% just given by the duration measure, the new price for bond A is:This is slightly less than the actual price of $. The difference is $ – $ = $. Next, we use the convexity measure to see if we can account for the difference of %. We have: convexity measure (half years) =2232121212(1)(100/)11(1)(1)(1)n n n d P C Cn n n C y dy P y y y y y P ++⎡⎤⎡⎤+-⎡⎤=--+⎢⎥⎢⎥⎢⎥+++⎣⎦⎣⎦⎣⎦ For bond A, we add 100 basis points and get a yield of 9%. We now have C = $40, y = %, n = 4, and M = $1,000. NOTE. In part (a) we computed the actual bond price and got P = $. Prior to that, the price sold at par (P = $1,000) since the coupon rate and yield were then equal. Expressing numbers in terms of a $100 bond quote, we have:C = $4, y = , n = 4, and P = $. Inserting these numbers into our convexity measure formula gives: convexity measure (half years) =342562$412($4)44(5)(100$4/0.045)1116.93250.045(1.045)0.045(1.045)(1.045)$98.2062y ⎡⎤⎡⎤-=⎡⎤--+=⎢⎥⎢⎥⎢⎥⎣⎦⎣⎦⎣⎦ Adding the duration measure and the convexity measure, we get ?% + % = ?%. Recall the actual change in price is: ($ – $1,000) = ?$ and the actual percentage change in price is: ?$ / $1,000 = ? or approximately ?%. Using the ?% resulting from both the duration and convexity measures, we can estimate the new price for bond A. We have:Adding the duration measure and the convexity measure, we get ?% + % = ?%. Recall the actual change in price is: ($ – $1,000) = ?$ and the actual percentage change in price is: ?$ / $1,000 = ? or approximately ?%. Using the ?% resulting from both the duration and convexity measures, we can estimate the new price for bond A. We have:This is slightly more negative than the actual percentage decrease in price of %. The difference is %)-%)=%Using the %just given by the duration measure, the new price for Bond B is:This is slightly less than the actual price of $1,000. This difference is $1,000-$=$ We use the convexity measure to see if we can account for the difference of 00594%. We have:For Bond B, 100 basis points are added and get a yield of 9%. We now have C=$45, y=%, n=10, and M=$1,000. Note in part (a), we computed the actual bond price and got P=$1,000 since the coupon rate and yield were then equal. Prior to that, the price sold at P=$1,. Expressing numbers in terms of a $100 bond quote, we have C=$, , n=10 and P=$100. Inserting these numbers into our convexity measure formula gives: The convexity measure (in years)=Note. Dollar Convexity Measure=Convexity Measure (years) times P=($100)=$1,. The percentage price change due to convexity is 21()2dP convexity measure dy P = Inserting in the values, we get 21(77.8103)(0.01)0.000974632dP P == Thus, we have % increase in price when we adjust for convexity measure.Adding the duration measure and convexity measure, we get %+% equals %. Recall the actual change in price is ($1,000-$1,=-$ and the actual new price isFor Bond A. This is about the same as the actual price of $1,000. The difference is $1,$1,000=$. Thus, using the convexity measure along with the duration measure has narrowed the estimated price from a difference of -$ to $.(d) Comment on the accuracy of your results in parts b and c, and state why one approximation is closer to the actual price than the other.Answer:For bond A, the actual price is $. When we use the duration measure, we get a bond price of $ that is $ less than the actual price. When we use duration and convex measures together, we get a bond price of $. This is slightly more than the actual price of $. The difference is $ – $ = $. Thus, using the convexity measure along with the duration measure has narrowed the estimated price from a difference of ?$ to $. For bond B, the actual price is $1,000. When we use the duration measure, we get a bond price of $ that is $ less than the actual price. When we use duration and convex measures together, we get a bond price of $1,. This is slightly more than the actual price of $1,000. The difference is $1, – $1,000 = $. Thus, using the convexity measure along with the duration measure has narrowed the estimated price from a difference of ?$ to $As we see, using the duration and convexity measures together is more accurate. The reason is that adding the convexity measure to our estimate enables us to include the second derivative that corrects for the convexity of the price-yield relationship. More details are offered below. Duration (modified or dollar) attempts to estimate a convex relationship with a straight line (the tangent line). We can specify a mathematical relationship that provides a better approximation to the price change of the bond if the required yield changes. We do this by using the first two terms of a Taylor series to approximate the price change as follows:Dividing both sides of this equation by P to get the percentage price change givesus:The first term on the right-hand side of equation (1) is equation for the dollar price change based on dollar duration and is our approximation of the price change based on duration. In equation (2), the first term on the right-hand side is the approximate percentage change in price based on modified duration. The second term in equations (1) and (2) includes the second derivative of the price function for computing the value of a bond. It is the second derivative that is used as a proxy measure to correct for the convexity of the price-yield relationship. Market participants refer to the second derivative of bond price function as the dollar convexity measure of the bond. The second derivative divided by price is a measure of the percentage change in the price of the bond due to convexity and is referred to simply as the convexity measure. (e) Without working through calculations, indicate whether the duration of the two bonds would be higher or lower if the yield to maturity is 10% rather than 8%. Answer: Like term to maturity and coupon rate, the yield to maturity is a factor that influences price volatility. Ceteris paribus, the higher the yield level, the lower the price volatility. The same property holds for modified duration. Thus, a 10% yield to maturity will have both less volatility than an 8% yield to maturity and also a smaller duration. There is consistency between the properties of bond price volatility and the properties of modified duration. When all other factors are constant, a bond with a longer maturity will have greater price volatility. A property of modified duration is that when all other factors are constant, a bond with a longer maturity will have a greater modified duration. Also, all other factors being constant, a bond with a lower coupon rate will have greater bond price volatility. Also, generally, a bond with a lower coupon rate will have a greater modified duration. Thus, bonds with greater durations will greater price volatilities.4)Suppose a client observes the following two benchmark spreads for two bonds: Bond issue U rated A: 150 basis pointsBond issue V rated BBB: 135 basis pointsYour client is confused because he thought the lower-rated bond (bond V) should offer a higher benchmark spread than the higher-rated bond (bond U). Explain why the benchmark spread may be lower for bond U.5)The bid and ask yields for a Treasury bill were quoted by a dealer as % and %, respectively. Shouldn’t the bid yield be less than the ask yield, because the bid yield indicates how much the dealer is willing to pay and the ask yield is what the dealer is willing to sell the Treasury bill for?Answer:The higher bid means a lower price. So the dealer is willing to pay less than would be paid for the lower ask price. We illustrate this below. Given the yield on a bank discount basis (Yd), the price of a Treasury bill is found by first solving the formula for the dollar discount (D), as follows:The price is then Price = F-DFor the 100-day Treasury bill with a face value (F) of $100,000, if the yield on a bank discount basis (Yd) is quoted as %, D is equal to:Therefore, price = $100,000 – $1, = $98,. For the 100-day Treasury bill with a face value (F) of $100,000, if the yield on a bank discount basis (Yd) is quoted as %, D is equal to:Therefore, price is: P = F – D = $100,000 – $1, = $98,.Thus, the higher bid quote of % (compared to lower ask quote %) gives a lower selling price of $98, (compared to $98,. The % higher yield translates into a selling price that is $ lower. In general, the quoted yield on a bank discount basis is not a meaningful measure of the return from holding a Treasury bill, for two reasons. First, the measure is based on a face-value investment rather than on the actual dollar amount invested. Second, the yield is annualized according to a 360-day rather than a 365-day year, making it difficult to compare Treasury bill yields with Treasury notes and bonds, which pay interest on a 365-day basis. The use of 360 days for a year is a money market convention for some money market instruments, however. Despite its shortcomings as a measure of return, this is the method that dealers have adopted to quote Treasury bills. Many dealer quote sheets, and some reporting services, provide two other yield measures that attempt to make the quoted yield comparable to that for a coupon bond and other money market instruments.6)What is the difference between a cash-out refinancing and a rate-and-term refinancing?Answer:When a lender is evaluating an application from a borrower who is refinancing, the loan-to-value ratio (LTV) is dependent upon the requested amount of the new loan and the market value of the property as determined by an appraisal. When the loan amount requested exceeds the original loan amount, the transaction is referred to as a cash-out-refinancing. If instead, there is financing where the loan balance remains unchanged, the transaction is said to be a rate-and-term refinancing or no-cash refinancing. That is, the purpose of refinancing the loan is to either obtain a better note rate or change the term of the loan.7) Describe the cash flow of a mortgage pass-through security.Answer:The cash flow of a mortgage pass-through security depends on the cash flow of the underlying cash flow consists of monthly mortgage payments representing interest,the scheduled repayment of principal,and any prepayments.Payments are made to security holders each theamount nor the timing,however,of the cash flow from the pool of mortgages is identical to that of the cash flow passed through to investors.The monthly cash flow for a pass-through is less than the monthly cash flow of the underlying mortgages by an amount equal to servicing and other other fees are those charged by the issuer or guarantor of the pass-through for guaranteeing the coupon rage on a pass-through,called the pass-through coupon rate,is less than the mortgage rage on the underlying pool of mortgage loans by an amount equal to the servicing and guaranteeing feesThe timing of the cash flow,like the amount of the cash flow,is also monthly mortgage payment is due from each mortgagor on the first day of each month,but there is a delay in passing through the corresponding monthly cash flow to the length of the delay varies by the type of pass-through security.Because of prepayments,the cash flow of a pass-through is also not known with certainty.。
固定收益证券_课后习题与答案
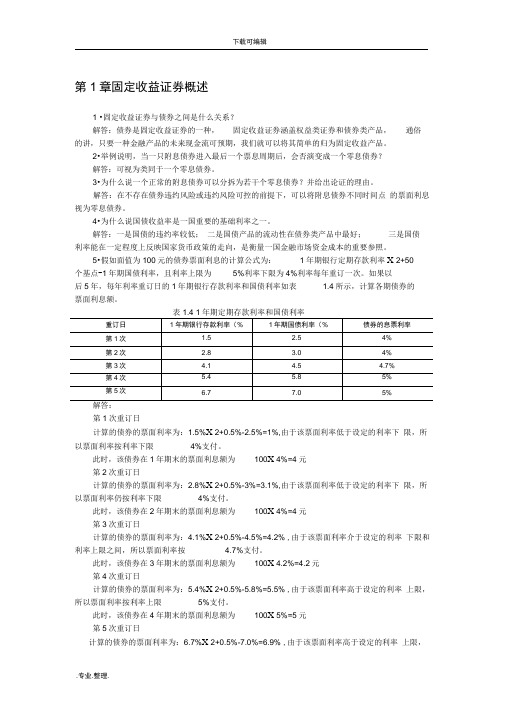
第1章固定收益证券概述1 •固定收益证券与债券之间是什么关系?解答:债券是固定收益证券的一种,固定收益证券涵盖权益类证券和债券类产品,通俗的讲,只要一种金融产品的未来现金流可预期,我们就可以将其简单的归为固定收益产品。
2•举例说明,当一只附息债券进入最后一个票息周期后,会否演变成一个零息债券?解答:可视为类同于一个零息债券。
3•为什么说一个正常的附息债券可以分拆为若干个零息债券?并给出论证的理由。
解答:在不存在债券违约风险或违约风险可控的前提下,可以将附息债券不同时间点的票面利息视为零息债券。
4•为什么说国债收益率是一国重要的基础利率之一。
解答:一是国债的违约率较低;二是国债产品的流动性在债券类产品中最好;三是国债利率能在一定程度上反映国家货币政策的走向,是衡量一国金融市场资金成本的重要参照。
5•假如面值为100元的债券票面利息的计算公式为:1年期银行定期存款利率X 2+50个基点-1年期国债利率,且利率上限为5%利率下限为4%利率每年重订一次。
如果以后5年,每年利率重订日的1年期银行存款利率和国债利率如表 1.4所示,计算各期债券的票面利息额。
第1次重订日计算的债券的票面利率为:1.5%X 2+0.5%-2.5%=1%,由于该票面利率低于设定的利率下限,所以票面利率按利率下限4%支付。
此时,该债券在1年期末的票面利息额为100X 4%=4元第2次重订日计算的债券的票面利率为:2.8%X 2+0.5%-3%=3.1%,由于该票面利率低于设定的利率下限,所以票面利率仍按利率下限4%支付。
此时,该债券在2年期末的票面利息额为100X 4%=4元第3次重订日计算的债券的票面利率为:4.1%X 2+0.5%-4.5%=4.2% ,由于该票面利率介于设定的利率下限和利率上限之间,所以票面利率按 4.7%支付。
此时,该债券在3年期末的票面利息额为100X 4.2%=4.2元第4次重订日计算的债券的票面利率为:5.4%X 2+0.5%-5.8%=5.5% ,由于该票面利率高于设定的利率上限,所以票面利率按利率上限5%支付。
2015固定收益证券《固定收益证券》课程作业

年 学 级: 2012 专 姓 业: 名: 金融工程 张永根 班 得 级: 金工 1201 班 分:
号:U201216614
1 (15 分)请简述债券投资者可能遭受的风险类型。 债券投资者可能面临的风险: (1)利率风险(2)信用风险(3)提前偿还风险(4)通货膨胀风险 (5)汇率风险(6)流动性风险(7)其他风险 2 (15 分)请简述债券评级机构的作用,并阐述你是如何看待债券评级机构的发展趋势的。 从理论上讲,债券评级机构至少发挥两个重要作用:提供信息和认证。 (1)债券市场上存在的信息不对称问题会导致逆向选择、道德风险等问题。由于存在 规模经济效应以及在收集、加工信息方面的专业化,评级机构在向投资者提供与债券违约 风险有关的信息的过程中能有效地降低信息搜寻成本。另外,评级机构能像债券发行公司 的利害相关者提供有价值的信息,降低其信息搜寻成本。 (2)政府机构对于某些金融机构的监管产生了对评级机构认证的需求。 当前我国债券信用评级还处于发展初期,存在评级质量不高、无序竞争等问题,难以 为投资者提供高质量、权威性的服务,阻碍了信用评级解决市场经济条件下的信息不对称 问题、防范信用风险、增强资本市场运作效率等作用的有效发挥。必须究其根本原因,解 决相应问题:一是加快征信立法进程,协调强化监管,推动建立社会信用体系,完善债券 市场评级外部环境;二是评级机构自身应完善内部管理,提高技术水平,加强自律合作, 努力树立行业公信力。 3 (15 分)什么是年金?普通年金与预付年金的主要区别是什么?假设投资者购买面值为 10000 元的 10 年期债券,年利率为 5%,每年末付息一次,第一次付息在一年之后。如果 投资者持有该债券直至到期日,将每年得到的利息以年利率 4%进行再投资,10 年后他一 共将获得多少资金?若该债券改为每年年初付息一次,其他条件不变,10 年后他一共将获 得多少资金? 年金是指在相同的间隔时间内陆续收到或付出相同金额的款项,例如,分期付款买房、 分期偿还贷款、发放养老金等,都属于年金收付的形式。 按照收付的时间,年金可以划分为以下两类: (1)普通年金(后付年金):指在各期期末收入或付出的年金。 (2)预付年金:指在各期期初收入或付出的年金。 解:每年末获得利息为:10000×5%=500(元)进行再投资将获得:
固定收益证券第六章 到期收益率与总收益分析

因此,对债券投资而言,通常是在监测相对静态的估值基础上,
利用久期和凸率等指标来测算其利率风险,因为组合中不同债券 的久期和凸率是可以加权平均的。
持有收益率与总收益分析
定义
持有期收益率是债券持有期间的收益率,其大小取决于债券资本 利得与再投资收益。
债券收益的来源
利息支付 利息收入的再投资收益 (再投资风险)
C = 票面利息 n = 至偿还期或者出售债券时利息支付次数 r =票面利息的再投资收益率
总收益分析
全部利息为 nC 利息的利息(利息的再投资收益)为:
(1 r ) n 1 C nC r
资本利得:
P n P 0
总收益分析(平价债券)
例1:假设投资者投资于面值为1000元,期限4年,票面利率
再投资收益率风险
性质1:若到期收益率曲线向右上方倾斜且保持不变,则投 资于长期零息债券是较优策略;或即使未来再投资收益率 上升,但只要不超过一定的幅度,投资于长期债券仍然是
相对有利的。
性质2:假设到期收益曲线为水平状,若该曲线在未来水平 向上移动,则投资短期零息债券是较优策略;若该曲线在 未来水平向下移动,则投资长期零息债券是较优策略。 性质3:若到期收益率曲线向下倾斜,要使长期债券获得与 短期债券相同的收益,市场利率必须下降。
根据债券定价原理,则A、B、C三种债券的价格分别为1018.686元 、1000元和950.623元。若再投资收益率相对于到期收益率水平下 降1%,则三只债券的持有期实际收益率分别为:
债券A:票面利息和本金为到期一次性收到,不存在再投 资风险,故 HPRA 6% 债券B:
1000 8% (1 5%) 1080 HPRB 1 7.89% 1000
固定收益证券题目及答案

二0、假设有一个债券,面值一00元,期限三年, 票面利率五%,每年付息一次,市场利率四%,试 计算其凸度.
二一、有一债券面值是一00元,初始到期收益 率为八%,修正久期是七.九五年,凸度是八四. 六0,债券价格是八四.九二七八元.当收益率下 降一00个基点时,试计算用修正久期预测的债 券价格和考虑凸度调整后的债券价格.
一八、试计算面值为一00元,到期收益率为五 %,期限为五年的贴现债券的久期和修正久期.
一九、有一债券,面值一00元,期限二0年,息票 利率八%,每年付息一次,到期收益率八%,价格 是一00元.当市场利率上升一0个基点时,市场 价格是九九.0二五四元;当市场利率下降一0 个基点时,市场价格是一00.九八九二元.求该 债券的有效久期.
一五、假设有三个不同期限债券,它们的数据 见下表,其中第一个为零息债券,后两个是附息
债券,且都是每年付息一次.试给出一年期到三
年期的即期收益率. 三个不同期限债券的数据
期限[年] 一 二 三
面值[元] 一00 一00 一00
息票利率[%]
0 五.四二 六.七八
市场价格[元] 九五.六0 一0二.三八 一0五.五六
二三、假设货币市场期限为三个月、六个月和九 个月的债券的实际季度收益率分别为0.七五%、 一.五%和二%,再假设该市场上存在期限为三个月 和九个月的两种贴现国债,面值都是一00元.如果 投资者的投资期限是三个月,并假定收益率曲线在 未来三个月里不会变化.请问该投资者应选择哪一 种债券投资?
《固定收益证券》课程练习题 及答案
一、某投资者在上海证券交易所市场上 以六%的年收益率申报买进二00手R00 三,请计算成交后的购回价[小数点后保留 三位].
二、设一家公司从员工工作第一年末开始,每 年给员工三000元福利存入一个银行账户,连 续存四年,三年期存款年复利率为六.五%,二年 期存款年复利率为五%,一年期存款年复利率 为三%,那么这个年金终值是多少?
固定收益证券-课后习题答案

第1章固定收益证券概述1.固定收益证券与债券之间是什么关系?解答:债券是固定收益证券的一种,固定收益证券涵盖权益类证券和债券类产品,通俗的讲,只要一种金融产品的未来现金流可预期,我们就可以将其简单的归为固定收益产品。
2.举例说明,当一只附息债券进入最后一个票息周期后,会否演变成一个零息债券?解答:可视为类同于一个零息债券。
3.为什么说一个正常的附息债券可以分拆为若干个零息债券?并给出论证的理由。
解答:在不存在债券违约风险或违约风险可控的前提下,可以将附息债券不同时间点的票面利息视为零息债券。
4.为什么说国债收益率是一国重要的基础利率之一。
解答:一是国债的违约率较低;二是国债产品的流动性在债券类产品中最好;三是国债利率能在一定程度上反映国家货币政策的走向,是衡量一国金融市场资金成本的重要参照。
5.假如面值为100元的债券票面利息的计算公式为:1年期银行定期存款利率×2+50个基点-1年期国债利率,且利率上限为5%,利率下限为4%,利率每年重订一次。
如果以后5年,每年利率重订日的1年期银行存款利率和国债利率如表1.4所示,计算各期债券的票面利息额。
表1.4 1年期定期存款利率和国债利率解答:第1次重订日计算的债券的票面利率为:1.5%×2+0.5%-2.5%=1%,由于该票面利率低于设定的利率下限,所以票面利率按利率下限4%支付。
此时,该债券在1年期末的票面利息额为100×4%=4元第2次重订日计算的债券的票面利率为:2.8%×2+0.5%-3%=3.1%,由于该票面利率低于设定的利率下限,所以票面利率仍按利率下限4%支付。
此时,该债券在2年期末的票面利息额为100×4%=4元第3次重订日计算的债券的票面利率为:4.1%×2+0.5%-4.5%=4.2%,由于该票面利率介于设定的利率下限和利率上限之间,所以票面利率按4.7%支付。
此时,该债券在3年期末的票面利息额为100×4.2%=4.2元第4次重订日计算的债券的票面利率为:5.4%×2+0.5%-5.8%=5.5%,由于该票面利率高于设定的利率上限,所以票面利率按利率上限5%支付。
固定收益证券(姚长辉)第六章作业参考答案

第六章1.由于期货合约的价格为95.25美元,因此,暗含的单利利率为(100-95.25)%=4.75%。
在到期日,利率变成了5.25%,期货合约的价格为94.75美元。
融资者出售期货合约,因此可以赚取1250美元,即(95.25-94.75)*2500=1250美元或者(525-475)*25=1250美元投资者在期货合约到期日可以获得这笔钱。
在2005年12月,融资者可以按照当期利率5.25%,借入100万美元,期限为90天。
90天之后他支付本息1013125美元,即1000000(1+0.0525(90)/360)=1013125而在2005年12月融资者收到的资金为1001000美元,其中1000美元是卖出欧洲美元期货合约获得的收入,即1000000+1000=1001000美元有效利率(基于360天)为4.744%,即1001000(1+r(90)/360)=1013125r=4.744%2.假定在0时点,2.5年期国债的到期收益率为5%,6个月回购协议的利率为4%(基于360的计息规则)。
债券价格为883854.29美元。
1000000/(1.025) (1.025) (1.025) (1.025) (1.025)=883854.29在0时点:订立6个月的回购协议,借入资金883854.29美元,以100万美元面值的债券为抵押品。
同时,在市场上购买面值100万美元的债权,价格为883854.29美元,并以此作为抵押品。
净现金流量为0。
6个月之后:偿还回购协议的借款,并支付利息:-883854.29(1+4%*180/360)=-901531.376美元;同时得到面值为100万美元的债券,期限为2年。
(1+y)(1+y)=1000000\901531.376y=5.32%3、方案一:T=0,买入期货合约,价格为X;卖标的债券得95元,并把所得的钱借出;T=0.5,买标的债券支付X+100*7%/2收回借出的钱,得本息95+95*5%/2如果不存在套利机会,则X+100*7%/2=95+95*5%/2X=93.875方案二:T=0,卖出期货合约,价格为Y;借钱95元,期限为6个月;T=0.5,买入债券支付Y+100*3.5%偿还本息95+95*4.5%如果不存在套利机会,则Y+100*3.5%=95+95*4.5%Y=95.775所以,期货交易的合理区间为93.875—95.775如果该债券的期货价格为97元,超过合理定价区间,则存在套利机会,T=0,卖出期货合约,价格为97元;借入资金95元购买债券;T=0.5 卖出债券得97+100*3.5%=100.5偿还本息 95+95*5%/2=99.275净收益为100.5-99.275=1.2254、(1)净利率变动1个百分点,则债券组合价值波动3.2个百分点(2)互换现金流(持续四年)你自己 BLIBOR+0.2%互换之所以降低了风险价值。
固定收益证券作业及答案

固定收益证券作业及答案1.三年后收到的100元现在的价值是多少?分别考虑复利20%、复利100%、复利0%、复利20%(半年计息)、复利20%(季计息)和复利20%(连续计息)的情况。
2.以连续复利方式计息,分别计算复利4%、复利20%(年计息)、复利20%(季计息)和复利100%的利率。
3.考虑以下问题:a。
___在交易日92年9月16日给出了票面利率为91/8's在92年12月31日到期,92年9月17日结算的政府债券,其标价为买入价101:23,卖出价101:25.求该债券的买入和卖出的收益率。
b。
在同一交易日,___对同时在92年12月31日到期和在92年9月17日结算的T-bill报出的买入和卖出折现率分别是2.88%和2.86%。
是否存在套利机会?(“买入”和“卖出”是从交易者的角度出发,你是以“买入价”卖出,以“卖出价”买入)4.在交易日92年9月16日,以10-26的价格买入了一张面值为2000万美元、到期日为2021年11月15日的STRIPs (零息债券)。
求该债券的到期收益率。
5.今天是1994年10月10日,星期一,是交易日。
以下是三种债券的相关信息:发行机构票面利率到期日到期收益___ 10% 8.00% 星期二,1/31/95费城(市政) 9% 7.00% 星期一,12/2/95___(机构) 8.50% 8% 星期五,7/28/95这三种债券的面值均为100美元,每半年付息一次。
注意到上表中最后一列是到期收益,它反映了给定到期日、某种特定债券的标准惯例。
在计算日期时,不考虑闰年,同时也要忽略假期。
回答以下问题时,需要写清楚计算过程,不能只是用计算器计算价格。
a。
计算___发行的国债的报价,假定该国债按照标准结算方式结算。
b。
计算费城发行的城市债券的报价,假定该债券的标准结算期为三天。
c。
计算___发行的机构债券的报价,假定该债券按照标准结算方式结算。
本题需要根据给定的到期收益曲线来计算固定付息债券的全价,以及在曲线上下移动100个基点时的全价。
固定收益证券6

《固定收益证券》综合测试题六一、单项选择题(每题2分,共计20分)1.假定到期收益率曲线是水平的,都是 5%。
一个债券票面利率为 6%,每年支付一次利息,期限 3年。
如果到期收益率曲线平行上升一个百分点,则债券价格变化()。
A.2.32B. 2.72C. 3.02D. 3.222. 某一8年期债券,第1~3年息票利率为6.5%,第4~5年为7%,第6 ~7年为7.5%,第8年升为8%就属于()A. 多级步高债券B. 递延债券C.区间债券D.棘轮债券3.在纯预期理论的条件下,先下降后上升的的收益率曲线表示:()A.对短期债券的需求下降,对长期债券的需求上升B.短期利率在未来被认为可能下降C. 对短期债券的需求上升,对长期债券的需求下降D.投资者有特殊的偏好4. 5年期债券的息票率为10%,当前到期收益率为8%,该债券的价格会()A.等于面值B.高于面值C.低于面值D.无法确定5.下面的风险衡量方法中,对含权债券利率风险的衡量最合适的是()。
A.麦考利久期B.有效久期C.修正久期D.凸度6. 债券组合管理采用的指数策略非常困难是()A.主要指数中包含的债券种类太多,很难按适当比例购买B.许多债券交易量很小,所以很难以一个公平的市场价格买到C.投资经理需要大量的管理工作A、B和C7. 债券的期限越长,其利率风险()。
A.越大B.越小C.与期限无关D.无法确定8. 一个投资者按 85 元的价格购买了面值为 100元的两年期零息债券。
投资者预计这两年的通货膨胀率将分别为 4%和 5%。
则该投资者购买这张债券的真实到期收益率为()。
A.3.8B.5.1C.2.5D.4.29. On-the-run债券与off-the-run债券存在不同,On-the-run债券()A.比off-the-run债券期限更短B.比off-the-run债券期限更长C.为公开交易,off-the-run债券则不然D. 是同类债券中最新发行的10. 一位投资经理说:“对债券组合进行单期免疫,仅需要满足以下两个条件:资产的久期和债务的久期相等;资产的现值与负债的现值相等。
固定收益证券题目及答案解读

3、一张期限为10年的等额摊还债券,每年等 额偿还的金额为100元;另有一张永久债券, 每年支付利息为50元。如果市场利率为8%, 试比较它们价格的大小。
4、若市场上有下表所示的两个债券,并假设 市场利率的波动率是10%,构建一个二期的利率 二叉树。 市场债券品种假设
品种 A 到期期限 息票利率 (年) (%) 1 3.50 折现率 (%) 3.50 当前价格 (元) 100.00
15、假设有3个不同期限债券,它们的数据 见下表,其中第一个为零息债券,后两个是附 息债券,且都是每年付息一次。试给出1年期 到3年期的即期收益率。 三个不同期限债券的数据
期限(年) 1 2 3 面值(元) 100 100 100 息票利率(%) 市场价格(元) 0 95.60 5.42 102.38 6.78 105.56
18、试计算面值为100元,到期收益率为5%, 期限为5年的贴现债券的久期和修正久期。
19、有一债券,面值100元,期限20年,息 票利率8%,每年付息一次,到期收益率8%, 价格是100元。当市场利率上升10个基点时, 市场价格是99.0254元;当市场利率下降10个 基点时,市场价格是100.9892元。求该债券 的有效久期。
20、假设有一个债券,面值100元,期限3年, 票面利率5%,每年付息一次,市场利率4%, 试计算其凸度。
21、有一债券面值是100元,初始到期收益率 为8%,修正久期是7.95年,凸度是84.60, 债券价格是84.9278元。当收益率下降100个 基点时,试计算用修正久期预测的债券价格 和考虑凸度调整后的债券价格。
9、有一附息债券,一年付息一次,期限5年, 票面金额为1000元,票面利率5.2%。某投资 者在该债券发行时以998元的发行价购入,持 满3年即以1002.20元的价格卖出。请计算该 投资者的持有期收益率是多少(可用简化公 式)?当期收益率有一企业债券,面值100元,期限3年, 票面利率4%,到期一次还本付息,利息所得 税税率为20%,请计算持有该债券到期的税 后复利到期收益率。
固定收益证券-课后习题答案

第1章固定收益证券概述1.固定收益证券与债券之间是什么关系?解答:债券是固定收益证券的一种,固定收益证券涵盖权益类证券和债券类产品,通俗的讲,只要一种金融产品的未来现金流可预期,我们就可以将其简单的归为固定收益产品。
2.举例说明,当一只附息债券进入最后一个票息周期后,会否演变成一个零息债券?解答:可视为类同于一个零息债券。
3.为什么说一个正常的附息债券可以分拆为若干个零息债券?并给出论证的理由。
解答:在不存在债券违约风险或违约风险可控的前提下,可以将附息债券不同时间点的票面利息视为零息债券。
4.为什么说国债收益率是一国重要的基础利率之一。
解答:一是国债的违约率较低;二是国债产品的流动性在债券类产品中最好;三是国债利率能在一定程度上反映国家货币政策的走向,是衡量一国金融市场资金成本的重要参照。
5.假如面值为100元的债券票面利息的计算公式为:1年期银行定期存款利率×2+50个基点-1年期国债利率,且利率上限为5%,利率下限为4%,利率每年重订一次。
如果以后5年,每年利率重订日的1年期银行存款利率和国债利率如表1.4所示,计算各期债券的票面利息额。
表1.4 1年期定期存款利率和国债利率解答:第1次重订日计算的债券的票面利率为:1.5%×2+0.5%-2.5%=1%,由于该票面利率低于设定的利率下限,所以票面利率按利率下限4%支付。
此时,该债券在1年期末的票面利息额为100×4%=4元第2次重订日计算的债券的票面利率为:2.8%×2+0.5%-3%=3.1%,由于该票面利率低于设定的利率下限,所以票面利率仍按利率下限4%支付。
此时,该债券在2年期末的票面利息额为100×4%=4元第3次重订日计算的债券的票面利率为:4.1%×2+0.5%-4.5%=4.2%,由于该票面利率介于设定的利率下限和利率上限之间,所以票面利率按4.7%支付。
此时,该债券在3年期末的票面利息额为100×4.2%=4.2元第4次重订日计算的债券的票面利率为:5.4%×2+0.5%-5.8%=5.5%,由于该票面利率高于设定的利率上限,所以票面利率按利率上限5%支付。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
第六次作业:《固定收益证券》
Page 263-264:3
3、有下面的互换:名义本金1亿元,互换期限5年,6个月互换1次。
做市商支付固定利率,为8%,同时收取浮动利率(按照6个月的LOBOR 收取)。
在很短的时间内,做市商无法冲销互换头寸。
他想利用债券市场避险,因此购买面值1亿元,期限5年,票面利率8%,平价交易的国债。
购买债券的资金来自于出售6个月的T-bill ,价格1亿元(6个月的到期收益率为5%)。
当互换合约签订后,很快利率下降,其中5年期债券的到期收益率降低到6%,6个月的到期收益率降低到3%。
请计算互换合约本身给做市商带来的损失。
如果做市商一周后找到了互换对手,可以对冲前面的互换,并假定一周内利率不再发生变化,请具体分析做市商的避险效果受哪些因素的影响。
答:(1)由题
*:由平价交易,所以到期收益率与票面利率相等。
当到期收益率下降时,债券价格上升,所以有
P1= 0.04
(1+0.03)n 10n =1+1(1+0.03)10=1.0853亿,因此做市商在5年期国债中,获得0.0853亿元收益。
因为利率下降至6%,而做市商支付固定利率8%,因此产生损失,每月为: 1×(8%-6%)/2=0.01亿
十年合计现值计算如下:
P2= 0.01
(1+0.03)n 10n =1=0.0853亿元
利率下降后,来自T-bill 的损失如下:
P3(1+6%/2)=1*(1+8%/2)
∴P3=1.0097亿元
所以,互换合约本身给做市商带来的损失为0.0853亿元,通过购买国债对冲风险,可以将损失降至0.0097亿元。
(2)由本题,做市商避险的效果受如下因素影响:
A.受避险产品的影响,很多避险产品不能完全满足避险需求;
B.受利率变化影响,利率期限结构变化无法预期;
C.受互换对手需求影响,是否有需求相对应的不同互换对手,通过做市商中介,本身可以对冲风险;
D.互换现金流的稳定性,要考虑不同条件变动时,互换现金流是否可以持续满足避险需求。
