半导体物理与器件第四版答案

合集下载

半导体物理与器件(尼曼第四版)答案

半导体物理与器件(尼曼第四版)答案第一章：半导体材料与晶体1.1 半导体材料的基本特性半导体材料是一种介于导体和绝缘体之间的材料。

它的基本特性包括：1.带隙：半导体材料的价带与导带之间存在一个禁带或带隙，是电子在能量上所能占据的禁止区域。

2.拉伸系统：半导体材料的结构是由原子或分子构成的晶格结构，其中的原子或分子以确定的方式排列。

3.载流子：在半导体中，存在两种载流子，即自由电子和空穴。

自由电子是在导带上的，在外加电场存在的情况下能够自由移动的电子。

空穴是在价带上的，当一个价带上的电子从该位置离开时，会留下一个类似电子的空位，空穴可以看作电子离开后的痕迹。

4.掺杂：为了改变半导体材料的导电性能，通常会对其进行掺杂。

掺杂是将少量元素添加到半导体材料中，以改变载流子浓度和导电性质。

1.2 半导体材料的结构与晶体缺陷半导体材料的结构包括晶体结构和非晶态结构。

晶体结构是指材料具有有序的周期性排列的结构，而非晶态结构是指无序排列的结构。

晶体结构的特点包括：1.晶体结构的基本单位是晶胞，晶胞在三维空间中重复排列。

2.晶格常数是晶胞边长的倍数，用于描述晶格的大小。

3.晶体结构可分为离子晶体、共价晶体和金属晶体等不同类型。

晶体结构中可能存在各种晶体缺陷，包括：1.点缺陷：晶体中原子位置的缺陷，主要包括实际缺陷和自间隙缺陷两种类型。

2.线缺陷：晶体中存在的晶面上或晶内的线状缺陷，主要包括位错和脆性断裂两种类型。

3.面缺陷：晶体中存在的晶面上的缺陷，主要包括晶面位错和穿孔两种类型。

1.3 半导体制备与加工半导体制备与加工是指将半导体材料制备成具有特定电性能的器件的过程。

它包括晶体生长、掺杂、薄膜制备和微电子加工等步骤。

晶体生长是将半导体材料从溶液或气相中生长出来的过程。

常用的晶体生长方法包括液相外延法、分子束外延法和气相外延法等。

掺杂是为了改变半导体材料的导电性能，通常会对其进行掺杂。

常用的掺杂方法包括扩散法、离子注入和分子束外延法等。

半导体物理与器件第四版课后习题标准答案

半导体物理与器件第四版课后习题答案————————————————————————————————作者：————————————————————————————————日期：2______________________________________________________________________________________3Chapter 33.1If o a were to increase, the bandgap energy would decrease and the material would beginto behave less like a semiconductor and morelike a metal. If o a were to decrease, the bandgap energy would increase and thematerial would begin to behave more like an insulator._______________________________________ 3.2Schrodinger's wave equation is:()()()t x x V x t x m ,,2222ψ⋅+∂ψ∂-η()tt x j ∂ψ∂=,ηAssume the solution is of the form:()()⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛-=ψt E kx j x u t x ηexp , Region I: ()0=x V . Substituting theassumed solution into the wave equation, we obtain:()⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛-⎩⎨⎧∂∂-t E kx j x jku x m ηηexp 22 ()⎪⎭⎪⎬⎫⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛-∂∂+t E kx j x x u ηexp()⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛-⋅⎪⎭⎫ ⎝⎛-=t E kx j x u jE j ηηηexp which becomes()()⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛-⎩⎨⎧-t E kx j x u jk m ηηexp 222()⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛-∂∂+t E kx j x x u jk ηexp 2()⎪⎭⎪⎬⎫⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛-∂∂+t E kx j x x u ηexp 22()⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛-+=t E kx j x Eu ηexp This equation may be written as()()()()0222222=+∂∂+∂∂+-x u mE x x u x x u jk x u k ηSetting ()()x u x u 1= for region I, the equation becomes: ()()()()021221212=--+x u k dx x du jk dxx u d α where222ηmE=αQ.E.D.In Region II, ()O V x V =. Assume the same form of the solution:()()⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛-=ψt E kx j x u t x ηexp , Substituting into Schrodinger's wave equation, we find:()()⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛-⎩⎨⎧-t E kx j x u jk m ηηexp 222()⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛-∂∂+t E kx j x x u jk ηexp 2()⎪⎭⎪⎬⎫⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛-∂∂+t E kx j x x u ηexp 22()⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛-+t E kx j x u V O ηexp()⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛-=t E kx j x Eu ηexp This equation can be written as:______________________________________________________________________________________4()()()2222xx u x x u jk x u k ∂∂+∂∂+- ()()02222=+-x u mEx u mV O ηη Setting ()()x u x u 2= for region II, this equation becomes()()dx x du jkdx x u d 22222+()022222=⎪⎪⎭⎫ ⎝⎛+--x u mV k O ηα where again222ηmE=αQ.E.D._______________________________________ 3.3We have ()()()()021221212=--+x u k dx x du jk dxx u d α Assume the solution is of the form: ()()[]x k j A x u -=αexp 1 ()[]x k j B +-+αexpThe first derivative is()()()[]x k j A k j dxx du --=ααexp 1 ()()[]x k j B k j +-+-ααexpand the second derivative becomes()()[]()[]x k j A k j dxx u d --=ααexp 2212()[]()[]x k j B k j +-++ααexp 2Substituting these equations into the differential equation, we find()()[]x k j A k ---ααexp 2()()[]x k j B k +-+-ααexp 2(){()[]x k j A k j jk --+ααexp 2()()[]}x k j B k j +-+-ααexp ()()[]{x k j A k ---ααexp 22 ()[]}0exp =+-+x k j B α Combining terms, we obtain()()()[]222222αααα----+--k k k k k ()[]x k j A -⨯αexp()()()[]222222αααα--++++-+k k k k k()[]0exp =+-⨯x k j B α We find that00= Q.E.D.For the differential equation in ()x u 2 and theproposed solution, the procedure is exactly the same as above._______________________________________ 3.4We have the solutions()()[]x k j A x u -=αexp 1()[]x k j B +-+αexp for a x <<0 and()()[]x k j C x u -=βexp 2()[]x k j D +-+βexp for 0<<-x b .The first boundary condition is ()()0021u u =which yields0=--+D C B AThe second boundary condition is201===x x dx dudx du which yields()()()C k B k A k --+--βαα ()0=++D k βThe third boundary condition is ()()b u a u -=21 which yields()[]()[]a k j B a k j A +-+-ααexp exp ()()[]b k j C --=βexp ()()[]b k j D -+-+βexp______________________________________________________________________________________5and can be written as()[]()[]a k j B a k j A +-+-ααexp exp ()[]b k j C ---βexp ()[]0exp =+-b k j D βThe fourth boundary condition isbx a x dx dudx du -===21 which yields()()[]a k j A k j --ααexp()()[]a k j B k j +-+-ααexp()()()[]b k j C k j ---=ββexp()()()[]b k j D k j -+-+-ββexp and can be written as()()[]a k j A k --ααexp()()[]a k j B k +-+-ααexp ()()[]b k j C k ----ββexp()()[]0exp =+++b k j D k ββ_______________________________________ 3.5(b) (i) First point: πα=aSecond point: By trial and error, πα729.1=a (ii) First point: πα2=aSecond point: By trial and error, πα617.2=a _______________________________________ 3.6(b) (i) First point: πα=aSecond point: By trial and error, πα515.1=a (ii) First point: πα2=aSecond point: By trial and error, πα375.2=a _______________________________________ 3.7ka a aaP cos cos sin =+'ααα Let y ka =, x a =α Theny x x xP cos cos sin =+'Consider dydof this function.()[]{}y x x x P dyd sin cos sin 1-=+⋅'- We find()()()⎭⎬⎫⎩⎨⎧⋅+⋅-'--dy dx x x dy dx x x P cos sin 112y dydx x sin sin -=-Theny x x x x x P dy dx sin sin cos sin 12-=⎭⎬⎫⎩⎨⎧-⎥⎦⎤⎢⎣⎡+-' For πn ka y ==,...,2,1,0=n 0sin =⇒y So that, in general,()()dkd ka d a d dy dxαα===0 And22ηmE=α SodkdEm mE dk d ⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛=-22/122221ηηα This implies thatdk dE dk d ==0α for an k π= _______________________________________ 3.8(a) πα=a 1π=⋅a E m o 212η______________________________________________________________________________________6()()()()2103123422221102.41011.9210054.12---⨯⨯⨯==ππa m E o η19104114.3-⨯=J From Problem 3.5 πα729.12=aπ729.1222=⋅a E m o η()()()()2103123422102.41011.9210054.1729.1---⨯⨯⨯=πE18100198.1-⨯=J12E E E -=∆1918104114.3100198.1--⨯-⨯= 19107868.6-⨯=Jor 24.4106.1107868.61919=⨯⨯=∆--E eV(b) πα23=aπ2223=⋅a E m o η()()()()2103123423102.41011.9210054.12---⨯⨯⨯=πE18103646.1-⨯=J From Problem 3.5, πα617.24=aπ617.2224=⋅a E m o η()()()()2103123424102.41011.9210054.1617.2---⨯⨯⨯=πE18103364.2-⨯=J34E E E -=∆1818103646.1103364.2--⨯-⨯= 1910718.9-⨯=Jor 07.6106.110718.91919=⨯⨯=∆--E eV_______________________________________ 3.9(a) At π=ka , πα=a 1π=⋅a E m o 212η()()()()2103123421102.41011.9210054.1---⨯⨯⨯=πE19104114.3-⨯=JAt 0=ka , By trial and error, πα859.0=a o()()()()210312342102.41011.9210054.1859.0---⨯⨯⨯=πo E19105172.2-⨯=J o E E E -=∆11919105172.2104114.3--⨯-⨯= 2010942.8-⨯=Jor 559.0106.110942.81920=⨯⨯=∆--E eV (b) At π2=ka , πα23=aπ2223=⋅a E m o η()()()()2103123423102.41011.9210054.12---⨯⨯⨯=πE18103646.1-⨯=J At π=ka . From Problem 3.5,πα729.12=aπ729.1222=⋅a E m o η()()()()2103123422102.41011.9210054.1729.1---⨯⨯⨯=πE18100198.1-⨯=J 23E E E -=∆1818100198.1103646.1--⨯-⨯= 19104474.3-⨯=Jor 15.2106.1104474.31919=⨯⨯=∆--E eV_____________________________________________________________________________________________________________________________73.10(a) πα=a 1π=⋅a E m o 212η()()()()2103123421102.41011.9210054.1---⨯⨯⨯=πE19104114.3-⨯=JFrom Problem 3.6, πα515.12=aπ515.1222=⋅a E m o η()()()()2103123422102.41011.9210054.1515.1---⨯⨯⨯=πE1910830.7-⨯=J 12E E E -=∆1919104114.310830.7--⨯-⨯= 19104186.4-⨯=Jor 76.2106.1104186.41919=⨯⨯=∆--E eV(b) πα23=aπ2223=⋅a E m o η()()()()2103123423102.41011.9210054.12---⨯⨯⨯=πE18103646.1-⨯=JFrom Problem 3.6, πα375.24=aπ375.2224=⋅a E m o η()()()()2103123424102.41011.9210054.1375.2---⨯⨯⨯=πE18109242.1-⨯=J 34E E E -=∆1818103646.1109242.1--⨯-⨯=1910597.5-⨯=Jor 50.3106.110597.51919=⨯⨯=∆--E eV _____________________________________3.11(a) At π=ka , πα=a 1π=⋅a E m o 212η()()()()2103123421102.41011.9210054.1---⨯⨯⨯=πE19104114.3-⨯=J At 0=ka , By trial and error, πα727.0=a o π727.022=⋅a E m o o η()()()()210312342102.41011.9210054.1727.0---⨯⨯⨯=πo E19108030.1-⨯=J o E E E -=∆11919108030.1104114.3--⨯-⨯= 19106084.1-⨯=Jor 005.1106.1106084.11919=⨯⨯=∆--E eV (b) At π2=ka , πα23=aπ2223=⋅a E m o η()()()()2103123423102.41011.9210054.12---⨯⨯⨯=πE18103646.1-⨯=JAt π=ka , From Problem 3.6, πα515.12=aπ515.1222=⋅a E m o η()()()()2103423422102.41011.9210054.1515.1---⨯⨯⨯=πE1910830.7-⨯=J 23E E E -=∆191810830.7103646.1--⨯-⨯=______________________________________________________________________________________81910816.5-⨯=Jor 635.3106.110816.51919=⨯⨯=∆--E eV_______________________________________ 3.12For 100=T K,()()⇒+⨯-=-1006361001073.4170.124g E164.1=g E eV200=T K, 147.1=g E eV 300=T K, 125.1=g E eV 400=T K, 097.1=g E eV 500=T K, 066.1=g E eV 600=T K, 032.1=g E eV _______________________________________ 3.13The effective mass is given by1222*1-⎪⎪⎭⎫ ⎝⎛⋅=dk E d m ηWe have()()B curve dkEd A curve dk E d 2222>so that ()()B curve m A curve m **<_______________________________________ 3.14The effective mass for a hole is given by1222*1-⎪⎪⎭⎫ ⎝⎛⋅=dk E d m p η We have that()()B curve dkEd A curve dk E d 2222> so that ()()B curve m A curve m p p **<_______________________________________ 3.15Points A,B: ⇒<0dkdEvelocity in -x direction Points C,D: ⇒>0dkdEvelocity in +x directionPoints A,D: ⇒<022dk Ednegative effective massPoints B,C: ⇒>022dkEd positive effective mass_______________________________________ 3.16For A: 2k C E i =At 101008.0+⨯=k m 1-, 05.0=E eV Or()()2119108106.105.0--⨯=⨯=E JSo ()2101211008.0108⨯=⨯-C3811025.1-⨯=⇒CNow ()()38234121025.1210054.12--*⨯⨯==C m η 311044.4-⨯=kgor o m m ⋅⨯⨯=--*31311011.9104437.4 o m m 488.0=*For B: 2k C E i =At 101008.0+⨯=k m 1-, 5.0=E eV Or ()()2019108106.15.0--⨯=⨯=E JSo ()2101201008.0108⨯=⨯-C 3711025.1-⨯=⇒CNow ()()37234121025.1210054.12--*⨯⨯==C m η321044.4-⨯=kgor o m m ⋅⨯⨯=--*31321011.9104437.4o m m 0488.0=*_____________________________________________________________________________________________________________________________93.17For A: 22k C E E -=-υ()()()2102191008.0106.1025.0⨯-=⨯--C3921025.6-⨯=⇒C ()()39234221025.6210054.12--*⨯⨯-=-=C m η31108873.8-⨯-=kgor o m m ⋅⨯⨯-=--*31311011.9108873.8o m m 976.0--=* For B: 22k C E E -=-υ()()()2102191008.0106.13.0⨯-=⨯--C382105.7-⨯=⇒C()()3823422105.7210054.12--*⨯⨯-=-=C m η3210406.7-⨯-=kgor o m m ⋅⨯⨯-=--*31321011.910406.7o m m 0813.0-=*_______________________________________ 3.18(a) (i) νh E =or ()()341910625.6106.142.1--⨯⨯==h E ν1410429.3⨯=Hz(ii) 141010429.3103⨯⨯===νλc E hc 51075.8-⨯=cm 875=nm(b) (i) ()()341910625.6106.112.1--⨯⨯==h E ν 1410705.2⨯=Hz(ii) 141010705.2103⨯⨯==νλc410109.1-⨯=cm 1109=nm _______________________________________ 3.19(c) Curve A: Effective mass is a constantCurve B: Effective mass is positive around 0=k , and is negativearound 2π±=k ._______________________________________ 3.20()[]O O k k E E E --=αcos 1 Then()()()[]O k k E dkdE---=ααsin 1()[]O k k E -+=ααsin 1 and()[]O k k E dkEd -=ααcos 2122Then221222*11ηηαE dk Ed m o k k =⋅== or212*αE m η=_______________________________________ 3.21(a) ()[]3/123/24l t dnm m m =*()()[]3/123/264.1082.04o o m m =o dnm m 56.0=*(b)oo l t cn m m m m m 64.11082.02123+=+=*oo m m 6098.039.24+=o cnm m 12.0=*_______________________________________ 3.22(a) ()()[]3/22/32/3lh hh dp m m m +=*______________________________________________________________________________________10()()[]3/22/32/3082.045.0o o m m +=[]o m ⋅+=3/202348.030187.0o dpm m 473.0=*(b) ()()()()2/12/12/32/3lh hh lh hh cpm m m m m ++=* ()()()()om ⋅++=2/12/12/32/3082.045.0082.045.0 o cpm m 34.0=*_______________________________________3.23For the 3-dimensional infinite potential well, ()0=x V when a x <<0, a y <<0, and a z <<0. In this region, the wave equation is:()()()222222,,,,,,z z y x y z y x x z y x ∂∂+∂∂+∂∂ψψψ()0,,22=+z y x mEψηUse separation of variables technique, so let ()()()()z Z y Y x X z y x =,,ψSubstituting into the wave equation, we have222222zZXY y Y XZ x X YZ ∂∂+∂∂+∂∂ 022=⋅+XYZ mEηDividing by XYZ , we obtain021*********=+∂∂⋅+∂∂⋅+∂∂⋅ηmE z Z Z y Y Y x X X Let01222222=+∂∂⇒-=∂∂⋅X k x X k x X X xx The solution is of the form: ()x k B x k A x X x x cos sin += Since ()0,,=z y x ψ at 0=x , then ()00=Xso that 0=B .Also, ()0,,=z y x ψ at a x =, so that()0=a X . Then πx x n a k = where...,3,2,1=x n Similarly, we have2221y k y Y Y -=∂∂⋅ and 2221z k zZ Z -=∂∂⋅From the boundary conditions, we find πy y n a k = and πz z n a k = where...,3,2,1=y n and ...,3,2,1=z n From the wave equation, we can write022222=+---ηmE k k k z y xThe energy can be written as()222222⎪⎭⎫ ⎝⎛++==a n n n m E E z y x n n n z y x πη _______________________________________ 3.24The total number of quantum states in the 3-dimensional potential well is given (in k-space) by()332a dk k dk k g T ⋅=ππ where222ηmEk =We can then writeηmEk 2=Taking the differential, we obtaindE Em dE E m dk ⋅⋅=⋅⋅⋅⋅=2112121ηηSubstituting these expressions into the densityof states function, we have()dE EmmE a dE E g T ⋅⋅⋅⎪⎭⎫ ⎝⎛=212233ηηππ Noting thatπ2h=ηthis density of states function can be simplified and written as______________________________________________________________________________________()()dE E m h a dE E g T ⋅⋅=2/33324πDividing by 3a will yield the density of states so that()()E hm E g ⋅=32/324π _______________________________________ 3.25For a one-dimensional infinite potential well,222222k an E m n ==*πη Distance between quantum states()()aa n a n k k n n πππ=⎪⎭⎫ ⎝⎛=⎪⎭⎫ ⎝⎛+=-+11Now()⎪⎭⎫ ⎝⎛⋅=a dkdk k g T π2NowE m k n*⋅=21ηdE Em dk n⋅⋅⋅=*2211η Then()dE Em a dE E g n T ⋅⋅⋅=*2212ηπDivide by the "volume" a , so()Em E g n *⋅=21πηSo()()()()()EE g 31341011.9067.0210054.11--⨯⋅⨯=π ()EE g 1810055.1⨯=m 3-J 1-_______________________________________ 3.26(a) Silicon, o nm m 08.1=*()()c nc E E h m E g -=*32/324π()dE E E h m g kTE E c nc c c⋅-=⎰+*232/324π()()kT E E c nc cE E h m 22/332/33224+*-⋅⋅=π()()2/332/323224kT h m n⋅⋅=*π ()()[]()()2/33342/33123210625.61011.908.124kT ⋅⋅⨯⨯=--π ()()2/355210953.7kT ⨯=(i) At 300=T K, 0259.0=kT eV ()()19106.10259.0-⨯=2110144.4-⨯=J Then()()[]2/3215510144.4210953.7-⨯⨯=c g25100.6⨯=m 3- or 19100.6⨯=c g cm 3-(ii) At 400=T K, ()⎪⎭⎫⎝⎛=3004000259.0kT034533.0=eV()()19106.1034533.0-⨯=21105253.5-⨯=J Then()()[]2/32155105253.5210953.7-⨯⨯=c g2510239.9⨯=m 3-or 191024.9⨯=c g cm 3-(b) GaAs, o nm m 067.0=*()()[]()()2/33342/33123210625.61011.9067.024kT g c ⋅⋅⨯⨯=--π ()()2/3542102288.1kT ⨯=______________________________________________________________________________________(i) At 300=T K, 2110144.4-⨯=kT J()()[]2/3215410144.42102288.1-⨯⨯=c g2310272.9⨯=m 3- or 171027.9⨯=c g cm 3-(ii) At 400=T K, 21105253.5-⨯=kT J()()[]2/32154105253.52102288.1-⨯⨯=c g2410427.1⨯=m 3- 181043.1⨯=c g cm 3-_______________________________________ 3.27(a) Silicon, o p m m 56.0=* ()()E E h mE g p-=*υυπ32/324()dE E E h m g E kTE p⋅-=⎰-*υυυυπ332/324()()υυυπE kTE pE E h m 32/332/33224-*-⎪⎭⎫ ⎝⎛-=()()[]2/332/333224kT h mp-⎪⎭⎫ ⎝⎛-=*π ()()[]()()2/33342/33133210625.61011.956.024kT ⎪⎭⎫ ⎝⎛⨯⨯=--π ()()2/355310969.2kT ⨯=(i)At 300=T K, 2110144.4-⨯=kT J ()()[]2/3215510144.4310969.2-⨯⨯=υg2510116.4⨯=m 3- or 191012.4⨯=υg cm 3-(ii)At 400=T K, 21105253.5-⨯=kT J ()()[]2/32155105253.5310969.2-⨯⨯=υg2510337.6⨯=m 3-or 191034.6⨯=υg cm 3- (b) GaAs, o p m m 48.0=*()()[]()()2/33342/33133210625.61011.948.024kT g ⎪⎭⎫ ⎝⎛⨯⨯=--πυ ()()2/3553103564.2kT ⨯=(i)At 300=T K, 2110144.4-⨯=kT J ()()[]2/3215510144.43103564.2-⨯⨯=υg2510266.3⨯=m 3- or 191027.3⨯=υg cm 3-(ii)At 400=T K, 21105253.5-⨯=kT J()()[]2/32155105253.53103564.2-⨯⨯=υg2510029.5⨯=m 3-or 191003.5⨯=υg cm 3-_______________________________________ 3.28(a) ()()c nc E E h m E g -=*32/324π()()[]()c E E -⨯⨯=--3342/33110625.61011.908.124πc E E -⨯=56101929.1 For c E E =; 0=c g1.0+=c E E eV; 4610509.1⨯=c g m 3-J 1-2.0+=c E E eV;4610134.2⨯=m 3-J 1- 3.0+=c E E eV; 4610614.2⨯=m 3-J 1- 4.0+=c E E eV; 4610018.3⨯=m 3-J 1-(b) ()E E hm g p-=*υυπ32/324()()[]()E E -⨯⨯=--υπ3342/33110625.61011.956.024E E -⨯=υ55104541.4______________________________________________________________________________________For υE E =; 0=υg 1.0-=υE E eV; 4510634.5⨯=υg m 3-J 1-2.0-=υE E eV;4510968.7⨯=m 3-J 1-3.0-=υE E eV; 4510758.9⨯=m 3-J 1-4.0-=υE E eV;4610127.1⨯=m 3-J 1-_______________________________________ 3.29(a) ()()68.256.008.12/32/32/3=⎪⎭⎫ ⎝⎛==**pnc m m g g υ(b) ()()0521.048.0067.02/32/32/3=⎪⎭⎫ ⎝⎛==**pncmm g g υ_______________________________________3.30 Plot_______________________________________ 3.31(a) ()()()!710!7!10!!!-=-=i i i i i N g N g W()()()()()()()()()()()()1201238910!3!7!78910===(b) (i) ()()()()()()()()12!10!101112!1012!10!12=-=i W 66= (ii)()()()()()()()()()()()()1234!8!89101112!812!8!12=-=i W 495=_______________________________________ 3.32()⎪⎪⎭⎫ ⎝⎛-+=kT E E E f F exp 11(a) kT E E F =-, ()()⇒+=1exp 11E f ()269.0=E f(b) kT E E F 5=-, ()()⇒+=5exp 11E f()31069.6-⨯=E f (c) kT E E F 10=-, ()()⇒+=10exp 11E f()51054.4-⨯=E f_______________________________________ 3.33()⎪⎪⎭⎫ ⎝⎛-+-=-kT E E E f F exp 1111or()⎪⎪⎭⎫⎝⎛-+=-kT E E E f F exp 111(a) kT E E F =-, ()269.01=-E f (b) kT E E F 5=-, ()31069.61-⨯=-E f (c) kT E E F 10=-, ()51054.41-⨯=-E f_______________________________________ 3.34(a) ()⎥⎦⎤⎢⎣⎡--≅kT E E f F F exp c E E =; 61032.90259.030.0exp -⨯=⎥⎦⎤⎢⎣⎡-=F f 2kT E c +; ()⎥⎦⎤⎢⎣⎡+-=0259.020259.030.0exp F f 61066.5-⨯=kT E c +; ()⎥⎦⎤⎢⎣⎡+-=0259.00259.030.0exp F f 61043.3-⨯=23kT E c +; ()()⎥⎦⎤⎢⎣⎡+-=0259.020259.0330.0exp F f 61008.2-⨯=kT E c 2+; ()()⎥⎦⎤⎢⎣⎡+-=0259.00259.0230.0exp F f 61026.1-⨯=______________________________________________________________________________________(b) ⎥⎦⎤⎢⎣⎡-+-=-kT E E f F F exp 1111()⎥⎦⎤⎢⎣⎡--≅kT E E F exp υE E =; ⎥⎦⎤⎢⎣⎡-=-0259.025.0exp 1F f 51043.6-⨯= 2kT E -υ; ()⎥⎦⎤⎢⎣⎡+-=-0259.020259.025.0exp 1F f 51090.3-⨯=kT E -υ; ()⎥⎦⎤⎢⎣⎡+-=-0259.00259.025.0exp 1F f 51036.2-⨯=23kT E -υ;()()⎥⎦⎤⎢⎣⎡+-=-0259.020259.0325.0exp 1F f 51043.1-⨯= kT E 2-υ;()()⎥⎦⎤⎢⎣⎡+-=-0259.00259.0225.0exp 1F f 61070.8-⨯=_______________________________________ 3.35()()⎥⎦⎤⎢⎣⎡-+-=⎥⎦⎤⎢⎣⎡--=kT E kT E kT E E f F c F F exp exp and()⎥⎦⎤⎢⎣⎡--=-kT E E f F F exp 1()()⎥⎦⎤⎢⎣⎡---=kT kT E E F υexp So ()⎥⎦⎤⎢⎣⎡-+-kT E kT E F c exp()⎥⎦⎤⎢⎣⎡+--=kT kT E E F υexp Then kT E E E kT E F F c +-=-+υOr midgap c F E E E E =+=2υ_______________________________________ 3.3622222man E n πη= For 6=n , Filled state()()()()()2103122234610121011.92610054.1---⨯⨯⨯=πE18105044.1-⨯=Jor 40.9106.1105044.119186=⨯⨯=--E eVFor 7=n , Empty state()()()()()2103122234710121011.92710054.1---⨯⨯⨯=πE1810048.2-⨯=Jor 8.12106.110048.219187=⨯⨯=--E eVTherefore 8.1240.9<<F E eV_______________________________________ 3.37(a) For a 3-D infinite potential well ()222222⎪⎭⎫⎝⎛++=a n n n mE z y x πη For 5 electrons, the 5thelectron occupies the quantum state 1,2,2===z y x n n n ; so()2222252⎪⎭⎫ ⎝⎛++=a n n n m E z y x πη()()()()()21031222223410121011.9212210054.1---⨯⨯++⨯=π 1910761.3-⨯=Jor 35.2106.110761.319195=⨯⨯=--E eV For the next quantum state, which is empty, the quantum state is 2,2,1===z y x n n n . This quantum state is at the same energy, so 35.2=F E eV(b) For 13 electrons, the 13th electronoccupies the quantum state______________________________________________________________________________________3,2,3===z y x n n n ; so ()()()()()2103122222341310121011.9232310054.1---⨯⨯++⨯=πE 1910194.9-⨯=Jor 746.5106.110194.9191913=⨯⨯=--E eVThe 14th electron would occupy the quantum state 3,3,2===z y x n n n . This state is at the same energy, so 746.5=F E eV_______________________________________ 3.38The probability of a state at E E E F ∆+=1 being occupied is()⎪⎭⎫ ⎝⎛∆+=⎪⎪⎭⎫ ⎝⎛-+=kT E kT E E E f F exp 11exp 11111 The probability of a state at E E E F ∆-=2being empty is()⎪⎪⎭⎫ ⎝⎛-+-=-kT E E E f F 222exp 1111⎪⎭⎫ ⎝⎛∆-+⎪⎭⎫ ⎝⎛∆-=⎪⎭⎫ ⎝⎛∆-+-=kT E kT E kT E exp 1exp exp 111 or()⎪⎭⎫ ⎝⎛∆+=-kT E E f exp 11122 so ()()22111E f E f -= Q.E.D._______________________________________ 3.39(a) At energy 1E , we want01.0exp 11exp 11exp 1111=⎪⎪⎭⎫ ⎝⎛-+⎪⎪⎭⎫ ⎝⎛-+-⎪⎪⎭⎫ ⎝⎛-kT E E kT E E kT E E F F FThis expression can be written as01.01exp exp 111=-⎪⎪⎭⎫ ⎝⎛-⎪⎪⎭⎫ ⎝⎛-+kT E E kT E E F For()⎪⎪⎭⎫⎝⎛-=kT E E F 1exp 01.01Then()100ln 1kT E E F += orkT E E F 6.41+= (b)At kT E E F 6.4+=,()()6.4exp 11exp 1111+=⎪⎪⎭⎫ ⎝⎛-+=kT E E E f F which yields()01.000990.01≅=E f_______________________________________ 3.40 (a)()()⎥⎦⎤⎢⎣⎡--=⎥⎦⎤⎢⎣⎡--=0259.050.580.5exp exp kT E E f F F 61032.9-⨯=(b) ()060433.03007000259.0=⎪⎭⎫⎝⎛=kT eV31098.6060433.030.0exp -⨯=⎥⎦⎤⎢⎣⎡-=F f (c) ()⎥⎦⎤⎢⎣⎡--≅-kT E E f F F exp 1 ⎥⎦⎤⎢⎣⎡-=kT 25.0exp 02.0______________________________________________________________________________________or 5002.0125.0exp ==⎥⎦⎤⎢⎣⎡+kT ()50ln 25.0=kTor()()⎪⎭⎫⎝⎛===3000259.0063906.050ln 25.0T kTwhich yields 740=T K_______________________________________ 3.41 (a)()00304.00259.00.715.7exp 11=⎪⎭⎫ ⎝⎛-+=E for 0.304%(b) At 1000=T K, 08633.0=kT eV Then()1496.008633.00.715.7exp 11=⎪⎭⎫ ⎝⎛-+=E for 14.96%(c) ()997.00259.00.785.6exp 11=⎪⎭⎫⎝⎛-+=E for 99.7% (d)At F E E =, ()21=E f for alltemperatures_______________________________________ 3.42(a) For 1E E =()()⎥⎦⎤⎢⎣⎡--≅⎪⎪⎭⎫ ⎝⎛-+=kT E E kTE E E fF F11exp exp 11Then()611032.90259.030.0exp -⨯=⎪⎭⎫ ⎝⎛-=E fFor 2E E =,82.030.012.12=-=-E E F eV Then()⎪⎭⎫ ⎝⎛-+-=-0259.082.0exp 1111E for()⎥⎦⎤⎢⎣⎡⎪⎭⎫ ⎝⎛---≅-0259.082.0exp 111E f141078.10259.082.0exp -⨯=⎪⎭⎫ ⎝⎛-=(b) For 4.02=-E E F eV, 72.01=-F E E eV At 1E E =,()()⎪⎭⎫⎝⎛-=⎥⎦⎤⎢⎣⎡--=0259.072.0exp exp 1kT E E E f F or()131045.8-⨯=E f At 2E E =,()()⎥⎦⎤⎢⎣⎡--=-kT E E E f F 2exp 1⎪⎭⎫ ⎝⎛-=0259.04.0expor()71096.11-⨯=-E f_______________________________________ 3.43(a) At 1E E =()()⎪⎭⎫⎝⎛-=⎥⎦⎤⎢⎣⎡--=0259.030.0exp exp 1kT E E E f F or()61032.9-⨯=E f At 2E E =, 12.13.042.12=-=-E E F eV So()()⎥⎦⎤⎢⎣⎡--=-kT E E E f F 2exp 1⎪⎭⎫ ⎝⎛-=0259.012.1exp______________________________________________________________________________________or()191066.11-⨯=-E f(b) For 4.02=-E E F ,02.11=-F E E eV At 1E E =,()()⎪⎭⎫⎝⎛-=⎥⎦⎤⎢⎣⎡--=0259.002.1exp exp 1kT E E E f F or()181088.7-⨯=E f At 2E E =,()()⎥⎦⎤⎢⎣⎡--=-kT E E E f F 2exp 1⎪⎭⎫ ⎝⎛-=0259.04.0expor ()71096.11-⨯=-E f_______________________________________ 3.44()1exp 1-⎥⎦⎤⎢⎣⎡⎪⎪⎭⎫ ⎝⎛-+=kTE E E f Fso()()2exp 11-⎥⎦⎤⎢⎣⎡⎪⎪⎭⎫ ⎝⎛-+-=kT E E dE E df F⎪⎪⎭⎫ ⎝⎛-⎪⎭⎫⎝⎛⨯kT E E kT F exp 1 or()2exp 1exp 1⎥⎦⎤⎢⎣⎡⎪⎪⎭⎫ ⎝⎛-+⎪⎪⎭⎫ ⎝⎛-⎪⎭⎫⎝⎛-=kT E E kT E E kT dE E df F F (a) At 0=T K, For()00exp =⇒=∞-⇒<dE dfE E F()0exp =⇒+∞=∞+⇒>dEdfE E FAt -∞=⇒=dEdfE E F(b) At 300=T K, 0259.0=kT eVFor F E E <<,0=dE dfFor F E E >>, 0=dEdfAt F E E =,()()65.91110259.012-=+⎪⎭⎫ ⎝⎛-=dE df (eV)1-(c) At 500=T K, 04317.0=kT eVFor F E E <<, 0=dE dfFor F E E >>, 0=dEdfAt F E E =, ()()79.511104317.012-=+⎪⎭⎫ ⎝⎛-=dE df (eV)1- _______________________________________ 3.45(a) At midgap E E =,()⎪⎪⎭⎫ ⎝⎛+=⎪⎪⎭⎫ ⎝⎛-+=kTE kTE E E f gF2exp 11exp 11Si: 12.1=g E eV, ()()⎥⎦⎤⎢⎣⎡+=0259.0212.1exp 11E for()101007.4-⨯=E fGe: 66.0=g E eV______________________________________________________________________________________()()⎥⎦⎤⎢⎣⎡+=0259.0266.0exp 11E for()61093.2-⨯=E f GaAs: 42.1=g E eV ()()⎥⎦⎤⎢⎣⎡+=0259.0242.1exp 11E for()121024.1-⨯=E f(b) Using the results of Problem 3.38, the answers to part (b) are exactly the same as those given in part (a)._______________________________________ 3.46(a) ()⎥⎦⎤⎢⎣⎡--=kT E E f F F exp ⎥⎦⎤⎢⎣⎡-=-kT 60.0exp 108 or ()810ln 60.0+=kT()032572.010ln 60.08==kT eV()⎪⎭⎫⎝⎛=3000259.0032572.0Tso 377=T K(b) ⎥⎦⎤⎢⎣⎡-=-kT 60.0exp 106()610ln 60.0+=kT()043429.010ln 60.06==kT ()⎪⎭⎫⎝⎛=3000259.0043429.0Tor 503=T K_______________________________________ 3.47(a) At 200=T K,()017267.03002000259.0=⎪⎭⎫⎝⎛=kT eV⎪⎪⎭⎫ ⎝⎛-+==kTE E f FF exp 1105.019105.01exp =-=⎪⎪⎭⎫⎝⎛-kT E E F()()()19ln 017267.019ln ==-kT E E F 05084.0=eV By symmetry, for 95.0=F f , 05084.0-=-F E E eVThen ()1017.005084.02==∆E eV (b) 400=T K, 034533.0=kT eV For 05.0=F f , from part (a),()()()19ln 034533.019ln ==-kT E E F 10168.0=eV Then ()2034.010168.02==∆E eV_______________________________________。

半导体物理与器件第四课后习题答案3.doc

Chapter 33.1If o a were to increase, the bandgap energy would decrease and the material would begin to behave less like a semiconductor and more like a metal. If o a were to decrease, the bandgap energy would increase and thematerial would begin to behave more like an insulator._______________________________________ 3.2Schrodinger's wave equation is:()()()t x x V xt x m ,,2222ψ⋅+∂ψ∂- ()tt x j ∂ψ∂=, Assume the solution is of the form:()()⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛-=ψt E kx j x u t x exp , Region I: ()0=x V . Substituting theassumed solution into the wave equation, we obtain:()⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛-⎩⎨⎧∂∂-t E kx j x jku x m exp 22 ()⎪⎭⎪⎬⎫⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛-∂∂+t E kx j x x u exp ()⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛-⋅⎪⎭⎫ ⎝⎛-=t E kx j x u jE j exp which becomes()()⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛-⎩⎨⎧-t E kx j x u jk m exp 222 ()⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛-∂∂+t E kx j x x u jkexp 2 ()⎪⎭⎪⎬⎫⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛-∂∂+t E kx j x x u exp 22 ()⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛-+=t E kx j x Eu exp This equation may be written as()()()()0222222=+∂∂+∂∂+-x u mE x x u x x u jk x u kSetting ()()x u x u 1= for region I, the equation becomes:()()()()021221212=--+x u k dx x du jk dxx u d α where222mE=αIn Region II, ()O V x V =. Assume the same form of the solution:()()⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛-=ψt E kx j x u t x exp , Substituting into Schrodinger's wave equation, we find:()()⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛-⎩⎨⎧-t E kx j x u jk m exp 222 ()⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛-∂∂+t E kx j x x u jkexp 2 ()⎪⎭⎪⎬⎫⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛-∂∂+t E kx j x x u exp 22 ()⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛-+t E kx j x u V O exp ()⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛-=t E kx j x Eu exp This equation can be written as:()()()2222x x u x x u jk x u k ∂∂+∂∂+- ()()02222=+-x u mEx u mV OSetting ()()x u x u 2= for region II, this equation becomes()()dx x du jk dxx u d 22222+ ()022222=⎪⎪⎭⎫ ⎝⎛+--x u mV k O α where again222mE=α_______________________________________3.3We have()()()()021221212=--+x u k dx x du jk dxx u d α Assume the solution is of the form: ()()[]x k j A x u -=αexp 1()[]x k j B +-+αexp The first derivative is()()()[]x k j A k j dxx du --=ααexp 1 ()()[]x k j B k j +-+-ααexp and the second derivative becomes()()[]()[]x k j A k j dxx u d --=ααexp 2212 ()[]()[]x k j B k j +-++ααexp 2Substituting these equations into the differential equation, we find()()[]x k j A k ---ααexp 2()()[]x k j B k +-+-ααexp 2(){()[]x k j A k j jk --+ααexp 2()()[]}x k j B k j +-+-ααexp ()()[]{x k j A k ---ααexp 22 ()[]}0exp =+-+x k j B α Combining terms, we obtain()()()[]222222αααα----+--k k k k k ()[]x k j A -⨯αexp()()()[]222222αααα--++++-+k k k k k ()[]0exp =+-⨯x k j B α We find that 00=For the differential equation in ()x u 2 and the proposed solution, the procedure is exactly the same as above._______________________________________ 3.4We have the solutions ()()[]x k j A x u -=αexp 1()[]x k j B +-+αexp for a x <<0 and()()[]x k j C x u -=βexp 2()[]x k j D +-+βexp for 0<<-x b .The first boundary condition is ()()0021u u =which yields0=--+D C B AThe second boundary condition is201===x x dx dudx du which yields()()()C k B k A k --+--βαα()0=++D k β The third boundary condition is ()()b u a u -=21 which yields()[]()[]a k j B a k j A +-+-ααexp exp ()()[]b k j C --=βexp()()[]b k j D -+-+βexp and can be written as()[]()[]a k j B a k j A +-+-ααexp exp ()[]b k j C ---βexp()[]0exp =+-b k j D β The fourth boundary condition isbx a x dx dudx du -===21 which yields()()[]a k j A k j --ααexp()()[]a k j B k j +-+-ααexp ()()()[]b k j C k j ---=ββexp()()()[]b k j D k j -+-+-ββexp and can be written as ()()[]a k j A k --ααexp()()[]a k j B k +-+-ααexp()()[]b k j C k ----ββexp()()[]0exp =+++b k j D k ββ_______________________________________ 3.5(b) (i) First point: πα=aSecond point: By trial and error, πα729.1=a (ii) First point: πα2=aSecond point: By trial and error, πα617.2=a_______________________________________3.6(b) (i) First point: πα=aSecond point: By trial and error, πα515.1=a (ii) First point: πα2=aSecond point: By trial and error, πα375.2=a_______________________________________ 3.7ka a aaP cos cos sin =+'αααLet y ka =, x a =α Theny x x xP cos cos sin =+'Consider dy dof this function.()[]{}y x x x P dyd sin cos sin 1-=+⋅'- We find()()()⎭⎬⎫⎩⎨⎧⋅+⋅-'--dy dx x x dy dx x x P cos sin 112y dydxx sin sin -=- Theny x x x x x P dy dx sin sin cos sin 12-=⎭⎬⎫⎩⎨⎧-⎥⎦⎤⎢⎣⎡+-'For πn ka y ==, ...,2,1,0=n 0sin =⇒y So that, in general,()()dk d ka d a d dy dxαα===0 And22 mE=αSodk dEm mE dk d ⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛=-22/122221 α This implies thatdk dE dk d ==0α for an k π= _______________________________________ 3.8(a) πα=a 1π=⋅a E m o 212()()()()2103123422221102.41011.9210054.12---⨯⨯⨯==ππa m E o19104114.3-⨯=J From Problem 3.5 πα729.12=aπ729.1222=⋅a E m o()()()()2103123422102.41011.9210054.1729.1---⨯⨯⨯=πE18100198.1-⨯=J 12E E E -=∆1918104114.3100198.1--⨯-⨯= 19107868.6-⨯=Jor 24.4106.1107868.61919=⨯⨯=∆--E eV(b) πα23=aπ2223=⋅a E m o()()()()2103123423102.41011.9210054.12---⨯⨯⨯=πE18103646.1-⨯=J From Problem 3.5, πα617.24=aπ617.2224=⋅a E m o()()()()2103123424102.41011.9210054.1617.2---⨯⨯⨯=πE18103364.2-⨯=J 34E E E -=∆1818103646.1103364.2--⨯-⨯= 1910718.9-⨯=Jor 07.6106.110718.91919=⨯⨯=∆--E eV_______________________________________3.9(a) At π=ka , πα=a 1π=⋅a E m o 212()()()()2103123421102.41011.9210054.1---⨯⨯⨯=πE19104114.3-⨯=JAt 0=ka , By trial and error, πα859.0=a o ()()()()210312342102.41011.9210054.1859.0---⨯⨯⨯=πoE19105172.2-⨯=J o E E E -=∆11919105172.2104114.3--⨯-⨯= 2010942.8-⨯=Jor 559.0106.110942.81920=⨯⨯=∆--E eV (b) At π2=ka , πα23=aπ2223=⋅a E m o()()()()2103123423102.41011.9210054.12---⨯⨯⨯=πE18103646.1-⨯=JAt π=ka . From Problem 3.5, πα729.12=aπ729.1222=⋅a E m o()()()()2103123422102.41011.9210054.1729.1---⨯⨯⨯=πE18100198.1-⨯=J23E E E -=∆1818100198.1103646.1--⨯-⨯= 19104474.3-⨯=Jor 15.2106.1104474.31919=⨯⨯=∆--E eV_______________________________________3.10(a) πα=a 1π=⋅a E m o 212()()()()2103123421102.41011.9210054.1---⨯⨯⨯=πE19104114.3-⨯=JFrom Problem 3.6, πα515.12=aπ515.1222=⋅a E m o()()()()2103123422102.41011.9210054.1515.1---⨯⨯⨯=πE1910830.7-⨯=J 12E E E -=∆1919104114.310830.7--⨯-⨯= 19104186.4-⨯=Jor 76.2106.1104186.41919=⨯⨯=∆--E eV (b) πα23=aπ2223=⋅a E m o()()()()2103123423102.41011.9210054.12---⨯⨯⨯=πE18103646.1-⨯=JFrom Problem 3.6, πα375.24=aπ375.2224=⋅a E m o()()()()2103123424102.41011.9210054.1375.2---⨯⨯⨯=πE18109242.1-⨯=J 34E E E -=∆1818103646.1109242.1--⨯-⨯= 1910597.5-⨯=Jor 50.3106.110597.51919=⨯⨯=∆--E eV_____________________________________3.11(a) At π=ka , πα=a 1π=⋅a E m o 212()()()()2103123421102.41011.9210054.1---⨯⨯⨯=πE19104114.3-⨯=JAt 0=ka , By trial and error, πα727.0=a oπ727.022=⋅a E m o o()()()()210312342102.41011.9210054.1727.0---⨯⨯⨯=πo E19108030.1-⨯=Jo E E E -=∆11919108030.1104114.3--⨯-⨯= 19106084.1-⨯=Jor 005.1106.1106084.11919=⨯⨯=∆--E eV (b) At π2=ka , πα23=aπ2223=⋅a E m o()()()()2103123423102.41011.9210054.12---⨯⨯⨯=πE18103646.1-⨯=JAt π=ka , From Problem 3.6,πα515.12=aπ515.1222=⋅a E m o()()()()2103423422102.41011.9210054.1515.1---⨯⨯⨯=πE1910830.7-⨯=J23E E E -=∆191810830.7103646.1--⨯-⨯= 1910816.5-⨯=Jor 635.3106.110816.51919=⨯⨯=∆--E eV_______________________________________3.12For 100=T K, ()()⇒+⨯-=-1006361001073.4170.124gE164.1=g E eV200=T K, 147.1=g E eV 300=T K, 125.1=g E eV 400=T K, 097.1=g E eV 500=T K, 066.1=g E eV 600=T K, 032.1=g E eV_______________________________________3.13The effective mass is given by1222*1-⎪⎪⎭⎫⎝⎛⋅=dk E d mWe have()()B curve dkE d A curve dk E d 2222> so that ()()B curve m A curve m **<_______________________________________ 3.14The effective mass for a hole is given by1222*1-⎪⎪⎭⎫ ⎝⎛⋅=dk E d m p We have that()()B curve dkEd A curve dk E d 2222> so that ()()B curve m A curve m p p **<_______________________________________ 3.15Points A,B: ⇒<0dk dEvelocity in -x directionPoints C,D: ⇒>0dk dEvelocity in +x directionPoints A,D: ⇒<022dk Ednegative effective massPoints B,C: ⇒>022dkEd positive effective mass _______________________________________3.16For A: 2k C E i =At 101008.0+⨯=k m 1-, 05.0=E eV Or ()()2119108106.105.0--⨯=⨯=E J So ()2101211008.0108⨯=⨯-C3811025.1-⨯=⇒CNow ()()38234121025.1210054.12--*⨯⨯==C m 311044.4-⨯=kgor o m m ⋅⨯⨯=--*31311011.9104437.4o m m 488.0=* For B: 2k C E i =At 101008.0+⨯=k m 1-, 5.0=E eV Or ()()2019108106.15.0--⨯=⨯=E JSo ()2101201008.0108⨯=⨯-C 3711025.1-⨯=⇒CNow ()()37234121025.1210054.12--*⨯⨯==C m 321044.4-⨯=kg or o m m ⋅⨯⨯=--*31321011.9104437.4o m m 0488.0=*_______________________________________ 3.17For A: 22k C E E -=-υ()()()2102191008.0106.1025.0⨯-=⨯--C 3921025.6-⨯=⇒C()()39234221025.6210054.12--*⨯⨯-=-=C m31108873.8-⨯-=kgor o m m ⋅⨯⨯-=--*31311011.9108873.8o m m 976.0--=* For B: 22k C E E -=-υ()()()2102191008.0106.13.0⨯-=⨯--C 382105.7-⨯=⇒C()()3823422105.7210054.12--*⨯⨯-=-=C m3210406.7-⨯-=kgor o m m ⋅⨯⨯-=--*31321011.910406.7o m m 0813.0-=*_______________________________________ 3.18(a) (i) νh E =or ()()341910625.6106.142.1--⨯⨯==h E ν1410429.3⨯=Hz(ii) 141010429.3103⨯⨯===νλc E hc 51075.8-⨯=cm 875=nm(b) (i) ()()341910625.6106.112.1--⨯⨯==h E ν1410705.2⨯=Hz(ii) 141010705.2103⨯⨯==νλc410109.1-⨯=cm 1109=nm_______________________________________ 3.19(c) Curve A: Effective mass is a constantCurve B: Effective mass is positive around 0=k , and is negativearound 2π±=k . _______________________________________ 3.20()[]O O k k E E E --=αcos 1 Then()()()[]O k k E dkdE ---=ααsin 1()[]O k k E -+=ααsin 1 and()[]O k k E dk E d -=ααcos 2122Then221222*11 αE dk Ed m o k k =⋅== or212*αE m =_______________________________________ 3.21(a) ()[]3/123/24lt dn m m m =*()()[]3/123/264.1082.04oom m =o dn m m 56.0=*(b)o o l t cnm m m m m 64.11082.02123+=+=*oo m m 6098.039.24+=o cn m m 12.0=*_______________________________________ 3.22(a) ()()[]3/22/32/3lh hh dp m m m +=*()()[]3/22/32/3082.045.0o om m +=[]o m ⋅+=3/202348.030187.0o dp m m 473.0=*(b) ()()()()2/12/12/32/3lh hh lh hh cpm m m m m ++=*()()()()om ⋅++=2/12/12/32/3082.045.0082.045.0 o cp m m 34.0=*_______________________________________ 3.23For the 3-dimensional infinite potential well, ()0=x V when a x <<0, a y <<0, and a z <<0. In this region, the wave equation is:()()()222222,,,,,,z z y x y z y x x z y x ∂∂+∂∂+∂∂ψψψ()0,,22=+z y x mEψ Use separation of variables technique, so let ()()()()z Z y Y x X z y x =,,ψSubstituting into the wave equation, we have222222zZXY y Y XZ x X YZ ∂∂+∂∂+∂∂ 022=⋅+XYZ mEDividing by XYZ , we obtain021*********=+∂∂⋅+∂∂⋅+∂∂⋅ mEz Z Z y Y Y x X XLet01222222=+∂∂⇒-=∂∂⋅X k x X k x X X xx The solution is of the form: ()x k B x k A x X x x cos sin +=Since ()0,,=z y x ψ at 0=x , then ()00=X so that 0=B .Also, ()0,,=z y x ψ at a x =, so that ()0=a X . Then πx x n a k = where ...,3,2,1=x n Similarly, we have2221y k y Y Y -=∂∂⋅ and 2221z k zZ Z -=∂∂⋅From the boundary conditions, we find πy y n a k = and πz z n a k =where...,3,2,1=y n and ...,3,2,1=z n From the wave equation, we can write022222=+---mE k k k z y xThe energy can be written as()222222⎪⎭⎫⎝⎛++==a n n n m E E z y x n n n z y x π _______________________________________ 3.24The total number of quantum states in the 3-dimensional potential well is given (in k-space) by()332a dk k dk k g T ⋅=ππ where222 mEk =We can then writemEk 2=Taking the differential, we obtaindE Em dE E m dk ⋅⋅=⋅⋅⋅⋅=2112121 Substituting these expressions into the density of states function, we have()dE E mmE a dE E g T ⋅⋅⋅⎪⎭⎫ ⎝⎛=212233 ππ Noting thatπ2h=this density of states function can be simplified and written as()()dE E m h a dE E g T ⋅⋅=2/33324π Dividing by 3a will yield the density of states so that()()E h m E g ⋅=32/324π _______________________________________ 3.25For a one-dimensional infinite potential well,222222k a n E m n ==*π Distance between quantum states()()aa n a n k k n n πππ=⎪⎭⎫ ⎝⎛=⎪⎭⎫ ⎝⎛+=-+11Now()⎪⎭⎫ ⎝⎛⋅=a dkdk k g T π2NowE m k n *⋅=21dE Em dk n⋅⋅⋅=*2211 Then()dE Em a dE E g n T ⋅⋅⋅=*2212 π Divide by the "volume" a , so ()Em E g n *⋅=21πSo()()()()()EE g 31341011.9067.0210054.11--⨯⋅⨯=π ()EE g 1810055.1⨯=m 3-J 1-_______________________________________ 3.26(a) Silicon, o n m m 08.1=*()()c nc E E h m E g -=*32/324π()dE E E h m g kTE E c nc c c⋅-=⎰+*232/324π()()kT E E c nc cE E h m 22/332/33224+*-⋅⋅=π()()2/332/323224kT hm n⋅⋅=*π ()()[]()()2/33342/33123210625.61011.908.124kT ⋅⋅⨯⨯=--π ()()2/355210953.7kT ⨯=(i) At 300=T K, 0259.0=kT eV()()19106.10259.0-⨯= 2110144.4-⨯=J Then ()()[]2/3215510144.4210953.7-⨯⨯=c g25100.6⨯=m 3-or 19100.6⨯=c g cm 3-(ii) At 400=T K, ()⎪⎭⎫⎝⎛=3004000259.0kT034533.0=eV()()19106.1034533.0-⨯= 21105253.5-⨯=J Then()()[]2/32155105253.5210953.7-⨯⨯=c g2510239.9⨯=m 3- or 191024.9⨯=c g cm 3-(b) GaAs, o nm m 067.0=*()()[]()()2/33342/33123210625.61011.9067.024kT g c ⋅⋅⨯⨯=--π ()()2/3542102288.1kT ⨯=(i) At 300=T K, 2110144.4-⨯=kT J ()()[]2/3215410144.42102288.1-⨯⨯=c g2310272.9⨯=m 3- or 171027.9⨯=c g cm 3-(ii) At 400=T K, 21105253.5-⨯=kT J ()()[]2/32154105253.52102288.1-⨯⨯=c g2410427.1⨯=m 3-181043.1⨯=c g cm 3-_______________________________________ 3.27(a) Silicon, o p m m 56.0=* ()()E E h mE g p-=*υυπ32/324()dE E E h mg E kTE p⋅-=⎰-*υυυυπ332/324()()υυυπE kTE pE E hm 32/332/33224-*-⎪⎭⎫ ⎝⎛-=()()[]2/332/333224kT hmp-⎪⎭⎫ ⎝⎛-=*π ()()[]()()2/33342/33133210625.61011.956.024kT ⎪⎭⎫ ⎝⎛⨯⨯=--π ()()2/355310969.2kT ⨯=(i)At 300=T K, 2110144.4-⨯=kT J ()()[]2/3215510144.4310969.2-⨯⨯=υg2510116.4⨯=m3-or 191012.4⨯=υg cm 3- (ii)At 400=T K, 21105253.5-⨯=kT J()()[]2/32155105253.5310969.2-⨯⨯=υg2510337.6⨯=m3-or 191034.6⨯=υg cm 3- (b) GaAs, o p m m 48.0=*()()[]()()2/33342/33133210625.61011.948.024kT g ⎪⎭⎫ ⎝⎛⨯⨯=--πυ ()()2/3553103564.2kT ⨯=(i)At 300=T K, 2110144.4-⨯=kT J()()[]2/3215510144.43103564.2-⨯⨯=υg2510266.3⨯=m 3- or 191027.3⨯=υg cm 3-(ii)At 400=T K, 21105253.5-⨯=kT J()()[]2/32155105253.53103564.2-⨯⨯=υg2510029.5⨯=m 3-or 191003.5⨯=υg cm 3-_______________________________________ 3.28(a) ()()c nc E E h m E g -=*32/324π()()[]()c E E -⨯⨯=--3342/33110625.61011.908.124πc E E -⨯=56101929.1 For c E E =; 0=c g1.0+=c E E eV; 4610509.1⨯=c g m 3-J 1-2.0+=c E E eV; 4610134.2⨯=m 3-J 1-3.0+=c E E eV; 4610614.2⨯=m 3-J 1- 4.0+=c E E eV; 4610018.3⨯=m 3-J 1- (b) ()E E h m g p-=*υυπ32/324()()[]()E E -⨯⨯=--υπ3342/33110625.61011.956.024E E -⨯=υ55104541.4 For υE E =; 0=υg1.0-=υE E eV; 4510634.5⨯=υg m 3-J 1-2.0-=υE E eV; 4510968.7⨯=m 3-J 1-3.0-=υE E eV; 4510758.9⨯=m 3-J 1-4.0-=υE E eV; 4610127.1⨯=m 3-J 1-_______________________________________ 3.29(a) ()()68.256.008.12/32/32/3=⎪⎭⎫ ⎝⎛==**pnc m m g g υ(b) ()()0521.048.0067.02/32/32/3=⎪⎭⎫ ⎝⎛==**pncmm g g υ_______________________________________3.30 Plot_______________________________________ 3.31(a) ()()()!710!7!10!!!-=-=i i i i i N g N g W()()()()()()()()()()()()1201238910!3!7!78910===(b) (i) ()()()()()()()()12!10!101112!1012!10!12=-=i W 66=(ii) ()()()()()()()()()()()()1234!8!89101112!812!8!12=-=i W 495=_______________________________________ 3.32()⎪⎪⎭⎫ ⎝⎛-+=kT E E E f F exp 11(a) kT E E F =-, ()()⇒+=1exp 11E f()269.0=E f (b) kT E E F 5=-, ()()⇒+=5exp 11E f()31069.6-⨯=E f(c) kT E E F 10=-, ()()⇒+=10exp 11E f ()51054.4-⨯=E f_______________________________________ 3.33()⎪⎪⎭⎫ ⎝⎛-+-=-kT E E E f F exp 1111or()⎪⎪⎭⎫ ⎝⎛-+=-kT E E E f F exp 111(a) kT E E F =-, ()269.01=-E f (b) kT E E F 5=-, ()31069.61-⨯=-E f(c) kT E E F 10=-, ()51054.41-⨯=-E f_______________________________________ 3.34(a) ()⎥⎦⎤⎢⎣⎡--≅kT E E f F F exp c E E =; 61032.90259.030.0exp -⨯=⎥⎦⎤⎢⎣⎡-=F f 2kT E c +; ()⎥⎦⎤⎢⎣⎡+-=0259.020259.030.0exp F f 61066.5-⨯=kT E c +; ()⎥⎦⎤⎢⎣⎡+-=0259.00259.030.0exp F f 61043.3-⨯=23kT E c +; ()()⎥⎦⎤⎢⎣⎡+-=0259.020259.0330.0exp F f 61008.2-⨯=kT E c 2+; ()()⎥⎦⎤⎢⎣⎡+-=0259.00259.0230.0exp F f 61026.1-⨯=(b) ⎥⎦⎤⎢⎣⎡-+-=-kT E E f F F exp 1111()⎥⎦⎤⎢⎣⎡--≅kT E E F exp υE E =; ⎥⎦⎤⎢⎣⎡-=-0259.025.0exp 1F f 51043.6-⨯= 2kT E -υ; ()⎥⎦⎤⎢⎣⎡+-=-0259.020259.025.0exp 1F f 51090.3-⨯=kT E -υ; ()⎥⎦⎤⎢⎣⎡+-=-0259.00259.025.0exp 1F f 51036.2-⨯=23kTE -υ; ()()⎥⎦⎤⎢⎣⎡+-=-0259.020259.0325.0exp 1F f 51043.1-⨯= kT E 2-υ;()()⎥⎦⎤⎢⎣⎡+-=-0259.00259.0225.0exp 1F f 61070.8-⨯=_______________________________________3.35()()⎥⎦⎤⎢⎣⎡-+-=⎥⎦⎤⎢⎣⎡--=kT E kT E kT E E f F c F F exp exp and()⎥⎦⎤⎢⎣⎡--=-kT E E f F F exp 1 ()()⎥⎦⎤⎢⎣⎡---=kT kT E E F υexp So ()⎥⎦⎤⎢⎣⎡-+-kT E kT E F c exp ()⎥⎦⎤⎢⎣⎡+--=kT kT E E F υexp Then kT E E E kT E F F c +-=-+υOr midgap c F E E E E =+=2υ_______________________________________ 3.3622222ma n E n π =For 6=n , Filled state()()()()()2103122234610121011.92610054.1---⨯⨯⨯=πE18105044.1-⨯=Jor 40.9106.1105044.119186=⨯⨯=--E eV For 7=n , Empty state ()()()()()2103122234710121011.92710054.1---⨯⨯⨯=πE1810048.2-⨯=Jor 8.12106.110048.219187=⨯⨯=--E eV Therefore 8.1240.9<<F E eV_______________________________________ 3.37(a) For a 3-D infinite potential well()222222⎪⎭⎫ ⎝⎛++=a n n n mE z y x π For 5 electrons, the 5th electron occupies the quantum state 1,2,2===z y x n n n ; so()2222252⎪⎭⎫ ⎝⎛++=a n n n m E z y x π()()()()()21031222223410121011.9212210054.1---⨯⨯++⨯=π1910761.3-⨯=Jor 35.2106.110761.319195=⨯⨯=--E eV For the next quantum state, which is empty, the quantum state is 2,2,1===z y x n n n . This quantum state is at the same energy, so 35.2=F E eV(b) For 13 electrons, the 13th electronoccupies the quantum state 3,2,3===z y x n n n ; so ()()()()()2103122222341310121011.9232310054.1---⨯⨯++⨯=πE 1910194.9-⨯=Jor 746.5106.110194.9191913=⨯⨯=--E eVThe 14th electron would occupy the quantum state 3,3,2===z y x n n n . This state is at the same energy, so 746.5=F E eV_______________________________________ 3.38The probability of a state at E E E F ∆+=1 being occupied is()⎪⎭⎫ ⎝⎛∆+=⎪⎪⎭⎫ ⎝⎛-+=kT E kT E E E f F exp 11exp 11111 The probability of a state at E E E F ∆-=2being empty is()⎪⎪⎭⎫ ⎝⎛-+-=-kT E E E f F 222exp 1111⎪⎭⎫ ⎝⎛∆-+⎪⎭⎫ ⎝⎛∆-=⎪⎭⎫ ⎝⎛∆-+-=kT E kT E kT E exp 1exp exp 111or()⎪⎭⎫ ⎝⎛∆+=-kT E E f exp 11122so ()()22111E f E f -=_______________________________________3.39(a) At energy 1E , we want01.0exp 11exp 11exp 1111=⎪⎪⎭⎫ ⎝⎛-+⎪⎪⎭⎫ ⎝⎛-+-⎪⎪⎭⎫ ⎝⎛-kT E E kT E E kT E E F F FThis expression can be written as01.01exp exp 111=-⎪⎪⎭⎫ ⎝⎛-⎪⎪⎭⎫ ⎝⎛-+kT E E kT E E F F or()⎪⎪⎭⎫⎝⎛-=kT E E F 1exp 01.01Then()100ln 1kT E E F += orkT E E F 6.41+= (b)At kT E E F 6.4+=, ()()6.4exp 11exp 1111+=⎪⎪⎭⎫ ⎝⎛-+=kT E E E f F which yields()01.000990.01≅=E f_______________________________________ 3.40 (a)()()⎥⎦⎤⎢⎣⎡--=⎥⎦⎤⎢⎣⎡--=0259.050.580.5exp exp kT E E f F F 61032.9-⨯=(b) ()060433.03007000259.0=⎪⎭⎫⎝⎛=kT eV31098.6060433.030.0exp -⨯=⎥⎦⎤⎢⎣⎡-=F f (c) ()⎥⎦⎤⎢⎣⎡--≅-kT E E f F F exp 1 ⎥⎦⎤⎢⎣⎡-=kT 25.0exp 02.0or 5002.0125.0exp ==⎥⎦⎤⎢⎣⎡+kT ()50ln 25.0=kTor()()⎪⎭⎫⎝⎛===3000259.0063906.050ln 25.0T kT which yields 740=T K_______________________________________ 3.41 (a)()00304.00259.00.715.7exp 11=⎪⎭⎫ ⎝⎛-+=E for 0.304%(b) At 1000=T K, 08633.0=kT eV Then()1496.008633.00.715.7exp 11=⎪⎭⎫ ⎝⎛-+=E for 14.96%(c) ()997.00259.00.785.6exp 11=⎪⎭⎫ ⎝⎛-+=E for 99.7% (d)At F E E =, ()21=E f for all temperatures_______________________________________ 3.42(a) For 1E E =()()⎥⎦⎤⎢⎣⎡--≅⎪⎪⎭⎫ ⎝⎛-+=kT E E kTE E E fF F11exp exp 11Then()611032.90259.030.0exp -⨯=⎪⎭⎫ ⎝⎛-=E fFor 2E E =, 82.030.012.12=-=-E E F eV Then()⎪⎭⎫ ⎝⎛-+-=-0259.082.0exp 1111E for()⎥⎦⎤⎢⎣⎡⎪⎭⎫ ⎝⎛---≅-0259.082.0exp 111E f141078.10259.082.0exp -⨯=⎪⎭⎫ ⎝⎛-=(b) For 4.02=-E E F eV,72.01=-F E E eVAt 1E E =,()()⎪⎭⎫⎝⎛-=⎥⎦⎤⎢⎣⎡--=0259.072.0exp exp 1kT E E E f F or()131045.8-⨯=E f At 2E E =,()()⎥⎦⎤⎢⎣⎡--=-kT E E E f F 2exp 1 ⎪⎭⎫ ⎝⎛-=0259.04.0expor()71096.11-⨯=-E f_______________________________________ 3.43(a) At 1E E =()()⎪⎭⎫⎝⎛-=⎥⎦⎤⎢⎣⎡--=0259.030.0exp exp 1kT E E E f F or()61032.9-⨯=E fAt 2E E =, 12.13.042.12=-=-E E F eV So()()⎥⎦⎤⎢⎣⎡--=-kT E E E f F 2exp 1 ⎪⎭⎫ ⎝⎛-=0259.012.1expor()191066.11-⨯=-E f (b) For 4.02=-E E F ,02.11=-F E E eV At 1E E =,()()⎪⎭⎫⎝⎛-=⎥⎦⎤⎢⎣⎡--=0259.002.1exp exp 1kT E E E f F or()181088.7-⨯=E f At 2E E =,()()⎥⎦⎤⎢⎣⎡--=-kT E E E f F 2exp 1 ⎪⎭⎫ ⎝⎛-=0259.04.0expor ()71096.11-⨯=-E f_______________________________________ 3.44()1exp 1-⎥⎦⎤⎢⎣⎡⎪⎪⎭⎫ ⎝⎛-+=kTE E E f Fso()()2exp 11-⎥⎦⎤⎢⎣⎡⎪⎪⎭⎫ ⎝⎛-+-=kT E E dE E df F⎪⎪⎭⎫ ⎝⎛-⎪⎭⎫⎝⎛⨯kT E E kT F exp 1or()2exp 1exp 1⎥⎦⎤⎢⎣⎡⎪⎪⎭⎫ ⎝⎛-+⎪⎪⎭⎫ ⎝⎛-⎪⎭⎫⎝⎛-=kT E E kT E E kT dE E df F F (a) At 0=T K, For()00exp =⇒=∞-⇒<dE dfE E F()0exp =⇒+∞=∞+⇒>dEdfE E FAt -∞=⇒=dEdfE E F(b) At 300=T K, 0259.0=kT eVFor F E E <<, 0=dE dfFor F E E >>, 0=dEdfAt F E E =,()()65.91110259.012-=+⎪⎭⎫ ⎝⎛-=dE df (eV)1-(c) At 500=T K, 04317.0=kT eVFor F E E <<, 0=dE dfFor F E E >>, 0=dEdfAt F E E =,()()79.511104317.012-=+⎪⎭⎫ ⎝⎛-=dE df (eV)1- _______________________________________ 3.45(a) At midgap E E =,()⎪⎪⎭⎫⎝⎛+=⎪⎪⎭⎫ ⎝⎛-+=kT E kTE E E f g F2exp 11exp 11Si: 12.1=g E eV, ()()⎥⎦⎤⎢⎣⎡+=0259.0212.1exp 11E for()101007.4-⨯=E fGe: 66.0=g E eV ()()⎥⎦⎤⎢⎣⎡+=0259.0266.0exp 11E for()61093.2-⨯=E f GaAs: 42.1=g E eV ()()⎥⎦⎤⎢⎣⎡+=0259.0242.1exp 11E for()121024.1-⨯=E f(b) Using the results of Problem 3.38, the answers to part (b) are exactly the same as those given in part (a)._______________________________________3.46(a) ()⎥⎦⎤⎢⎣⎡--=kT E E f F F exp ⎥⎦⎤⎢⎣⎡-=-kT 60.0exp 108or()810ln 60.0+=kT()032572.010ln 60.08==kT eV ()⎪⎭⎫⎝⎛=3000259.0032572.0Tso 377=T K(b) ⎥⎦⎤⎢⎣⎡-=-kT 60.0exp 106()610ln 60.0+=kT()043429.010ln 60.06==kT ()⎪⎭⎫⎝⎛=3000259.0043429.0Tor 503=T K_______________________________________ 3.47(a) At 200=T K,()017267.03002000259.0=⎪⎭⎫⎝⎛=kT eV⎪⎪⎭⎫ ⎝⎛-+==kT E E f F F exp 1105.019105.01exp =-=⎪⎪⎭⎫ ⎝⎛-kT E E F()()()19ln 017267.019ln ==-kT E E F 05084.0=eV By symmetry, for 95.0=F f , 05084.0-=-F E E eVThen ()1017.005084.02==∆E eV (b) 400=T K, 034533.0=kT eV For 05.0=F f , from part (a),()()()19ln 034533.019ln ==-kT E E F 10168.0=eVThen ()2034.010168.02==∆E eV _______________________________________。

《半导体物理与器件》第四版答案第13章

Chapter 1313.1Sketch_______________________________________ 13.2Sketch_______________________________________ 13.3(a) sdpO N ea V ∈=22(i)()()()()()141624191085.81.1321031040.0106.1---⨯⨯⨯⨯=pOV 312.3=V(ii) ()()()()⎥⎥⎦⎤⎢⎢⎣⎡⨯⨯⨯=261816108.1102103ln 0259.0bi V328.1=V312.3328.1-=-=pO bi p V V V984.1-=V(b) ()2/122⎥⎦⎤⎢⎣⎡-+∈=d GS DS bi s eN V V V h(i) 2h()()()()()()2/1161914103106.15.00328.11085.81.132⎥⎦⎤⎢⎣⎡⨯⨯--+⨯=-- 521097.2-⨯=h cm μ297.0=m μ103.0297.040.02=-=-h a m (ii) 2h()()()()()()2/1161914103106.15.05.0328.11085.81.132⎥⎦⎤⎢⎣⎡⨯⨯--+⨯=-- 521035.3-⨯=h cm μ335.0=m μ065.0335.040.02=-=-h a m (iii) 2h()()()()()()2/1161914103106.15.05.2328.11085.81.132⎥⎦⎤⎢⎣⎡⨯⨯--+⨯=-- 521057.4-⨯=h cm μ457.0=m 022=-⇒>h a a h(c) ()()GS bi pO DS V V V sat V --=(i)()()0328.1312.3--=sat V DS 984.1=V(ii) ()()()0.1328.1312.3---=sat V DS 984.0=V_______________________________________ 13.4(a) sdpO N ea V ∈=22(i)()()()()()141624191085.87.1121031040.0106.1---⨯⨯⨯⨯=pOV 709.3=V(ii) ()()()()⎥⎥⎦⎤⎢⎢⎣⎡⨯⨯⨯=2101816105.1102103ln 0259.0bi V860.0=V709.3860.0-=-=pO bi p V V V849.2-=V(b) ()2/122⎥⎦⎤⎢⎣⎡-+∈=d GS DS bi s eN V V V h(i) 2h()()()()()()2/1161914103106.15.00860.01085.87.112⎥⎦⎤⎢⎣⎡⨯⨯--+⨯=-- 521042.2-⨯=h cm μ242.0=m μ158.0242.040.02=-=-h a m (ii) 2h()()()()()()2/1161914103106.15.05.0860.01085.87.112⎥⎦⎤⎢⎣⎡⨯⨯--+⨯=-- 521083.2-⨯=h cm μ283.0=mμ117.0283.040.02=-=-h a m(iii) 2h()()()()()()2/1161914103106.15.05.2860.01085.87.112⎥⎦⎤⎢⎣⎡⨯⨯--+⨯=-- 521008.4-⨯=h cm μ408.0=m 022=-⇒>h a a h(c) ()()GS bi pO DS V V V sat V --=(i)()()0860.0705.3--=sat V DS845.2=V(ii) ()()()0.1860.0705.3---=sat V DS 845.1=V_______________________________________ 13.5(a) 2222ea V N N ea V pO s a s apO ∈=⇒∈= ()()()()()2419141065.0106.175.21085.81.132---⨯⨯⨯=a N1510433.9⨯=cm 3-(b) ()()()()⎥⎥⎦⎤⎢⎢⎣⎡⨯⨯=261815108.11010433.9ln 0259.0bi V 280.1=V280.175.2-=-=bi pO p V V V47.1=V(c) 2265.015.0h h a -==- μ50.02=h m()2/122⎥⎦⎤⎢⎣⎡++∈=a GS SD bi s eN V V V h()241050.0-⨯()()()()()⎥⎥⎦⎤⎢⎢⎣⎡⨯⨯++⨯=--15191410433.9106.1028.11085.81.132GSV()()GSV +⨯=⨯--28.1105363.1105.299347.0=⇒GS V V(d) ()2/122⎥⎦⎤⎢⎣⎡++∈=a GS SD bi s eN V V V h()241065.0-⨯()()()()()⎥⎥⎦⎤⎢⎢⎣⎡⨯⨯+⨯=--15191410433.9106.128.11085.81.132SD V ()()()SD V +⨯=⨯--28.1105363.11065.092447.1=⇒SD V V_______________________________________ 13.6(a) 22ea V N pOs a ∈=()()()()()2419141065.0106.175.21085.87.112---⨯⨯⨯=or a N 1510425.8⨯=cm 3- (b) ()()()()⎥⎥⎦⎤⎢⎢⎣⎡⨯⨯=2101815105.11010425.8ln 0259.0bi V 8095.0=V8095.075.2-=-=bi pO p V V V9405.1=V(c) 2265.015.0h h a -==- μ50.02=⇒h m()2/122⎥⎦⎤⎢⎣⎡++∈=a GS SD bi s eN V V V h()241050.0-⨯()()()()()⎥⎥⎦⎤⎢⎢⎣⎡⨯⨯++⨯=--15191410425.8106.108095.01085.87.112GS V ()()GS V +⨯=⨯--8095.010536.1105.299 8178.0=⇒GS V V(d) ()2/122⎥⎦⎤⎢⎣⎡++∈=a GS SD bi s eN V V V h()241065.0-⨯()()()()()⎥⎥⎦⎤⎢⎢⎣⎡⨯⨯+⨯=--15191410425.8106.18095.01085.87.112SDV()()()SDV +⨯=⨯--8095.010536.11065.092494.1=⇒SD V V_______________________________________ 13.7(a) ⎪⎪⎭⎫⎝⎛=2ln i d a t bi n N N V V ()()()()⎥⎥⎦⎤⎢⎢⎣⎡⨯⨯⨯=2101816105.1103102ln 0259.0860.0=V bi pO p V V V -=86.3860.00.3=⇒-=pO pO V V VNow 2/12⎥⎦⎤⎢⎣⎡∈=a pO s eN V a()()()()()2/1161914102106.186.31085.87.112⎥⎦⎤⎢⎣⎡⨯⨯⨯=--5100.5-⨯≅cm μ50.0=m(b) 86.3=pO V V(c) ()()GS bi pO SD V V V sat V +-=(i) ()0.386.086.3=-=sat V SD V (ii) ()()5.15.186.086.3=+-=sat V SD V _______________________________________13.8(a) ⎪⎪⎭⎫⎝⎛=2ln i d a t bi n N N V V()()()()⎥⎥⎦⎤⎢⎢⎣⎡⨯⨯⨯=261816108.1103102ln 0259.0 328.1=Vbi pO p V V V -=328.4328.10.3=⇒-=pO pO V V V2/12⎥⎦⎤⎢⎣⎡∈=a pO s eN V a()()()()()2/1161914102106.1328.41085.81.132⎥⎦⎤⎢⎣⎡⨯⨯⨯=-- 51060.5-⨯=cm μ560.0=m(b) 328.4=pO V V(c) ()()GS bi pO SD V V V sat V +-=(i) ()()0.30328.1328.4=+-=sat V SD V (ii) ()()5.15.1328.1328.4=+-=sat V SD V _______________________________________ 13.9(a) ()()GS bi pO DS V V V sat V --= Now()()()()⎥⎥⎦⎤⎢⎢⎣⎡⨯⨯⨯=2101816105.1104104ln 0259.0bi V886.0=V We find886.5886.05=⇒-=pO pO V V V2/12⎥⎦⎤⎢⎣⎡∈=a pO s eN V a()()()()()2/1161914104106.1886.51085.87.112⎥⎦⎤⎢⎣⎡⨯⨯⨯=--51036.4-⨯=cm μ436.0=m(b) (i) 886.5=pO V V(ii) 0.5886.5886.0-=-=-=pO bi p V V V V _______________________________________13.10 (a) ()()()()⎥⎥⎦⎤⎢⎢⎣⎡⨯⨯=261815108.110105ln 0259.0bi V 264.1=V()()GS bi pO SD V V V sat V +-= ()0.1264.15.3+-=pO V 764.5=⇒pO V V 2/12⎥⎦⎤⎢⎣⎡∈=a pO s eN V a ()()()()()2/1151914105106.1764.51085.81.132⎥⎦⎤⎢⎣⎡⨯⨯⨯=-- 410293.1-⨯=cm μ293.1=m (b) (i) 764.5=pO V V(ii) 264.1764.5-=-=bi pO p V V V 5.4=V _______________________________________13.11 (a) ()L W a eN I s d n P ∈=6321μ()()()[]()()14216191085.87.11610106.11000--⨯⨯=()()43441020105.010400---⨯⨯⨯⨯or03.11=P I mA (b)sdPO N ea V ∈=22()()()()()⎥⎥⎦⎤⎢⎢⎣⎡⨯⨯⨯=---141624191085.87.11210105.0106.1 or93.1=PO V V Also()()()()874.0105.11010ln 0259.02101619=⎥⎥⎦⎤⎢⎢⎣⎡⨯=bi V VNow()()GS bi PO DS V V V sat V --= GS V +-=874.093.1 or()GS DS V sat V +=056.1We have 056.193.1874.0-=-=-=PO bi P V V V V Then (i) For 0=GS V , ()06.1=sat V DS V (ii) For 264.041-==P GS V V V, ()792.0=sat V DS V (iii) For 528.021-==P GS V V V,()528.0=sat V DS V(iv) For 792.043-==P P V V V, ()264.0=sat V DS V (c)()⎢⎢⎣⎡⎪⎪⎭⎫⎝⎛--=PO GS bi P D V V V I sat I 3111 ⎥⎥⎦⎤⎪⎪⎭⎫ ⎝⎛--⨯PO GS bi V V V 321 ()⎢⎣⎡⎪⎪⎭⎫⎝⎛--=93.1874.03103.1GS V ⎥⎥⎦⎤⎪⎪⎭⎫ ⎝⎛--⨯93.1874.0321GS V (i) For 0=GS V , ()258.01=sat I D mA(ii) For 264.0-=GS V V,()141.01=sat I D mA(iii) For 528.0-=GS V V, ()0608.01=sat I D mA (iv) For 792.0-=GS V V,()0148.01=sat I D mA_______________________________________13.12⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛--=2/111PO GSbi O d V V V G g where()93.11003.133311-⨯==PO P O V I Gor 311060.1-⨯=O G S 60.1=mS Then13.13n-channel JFET - GaAs (a) LW aN e G d n O μ=1()()()4161910101028000106.1--⨯⨯⨯= ()()441035.01030--⨯⨯⨯ or 311069.2-⨯=O G S(b) ()()GS bi PO DS V V V sat V --= We havesd PO N ea V ∈=22 ()()()()()141624191085.81.1321021035.0106.1---⨯⨯⨯⨯= or 69.1=PO V V We find()()()()⎥⎥⎦⎤⎢⎢⎣⎡⨯⨯⨯=261618108.1102105ln 0259.0bi Vor 34.1=bi V V Then 35.069.134.1-=-=-=PO bi P V V V V We then obtain ()()GS GS DS V V sat V +=--=35.034.169.1 For 0=GS V , ()35.0=sat V DS V For 175.021-==P GS V V V, ()175.0=sat V DS V (c)()⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛--=PO GS bi P D V V V I sat I 3111 ⎥⎥⎦⎤⎪⎪⎭⎫ ⎝⎛--⨯POGS bi V V V 321where ()L W a eN I s d n P ∈=6321μ ()()()[]()()14216191085.81.136102106.18000--⨯⨯⨯=()()434410101035.01030---⨯⨯⨯⨯or 515.11=P I mA Then()()⎢⎣⎡⎪⎪⎭⎫⎝⎛--=69.134.131515.11GS D V sat I ⎥⎥⎦⎤⎪⎪⎭⎫ ⎝⎛--⨯69.134.1321GS V (mA) For 0=GS V , ()0506.01=sat I D mAandFor 175.0-=GS V V,()0124.01=sat I D mA_______________________________________13.14 ⎪⎪⎭⎫ ⎝⎛--=PO GS biPO P mS V V V V I g 131 We have03.11=P I mA, 93.1=PO V V, 874.0=bi V VThe maximum transconductance occurs when0=GS V . Then()()⎪⎪⎭⎫ ⎝⎛-=93.1874.0193.103.13m ax mS g or ()524.0max =mS g mS For μ400=W m, we have ()410400524.0m ax -⨯=mS gor()1.13max =mS g mS/cm = 1.31 mS/mm _______________________________________13.15The maximum transconductance occurs for 0=GS V , so we have (a) ()⎪⎪⎭⎫ ⎝⎛-=PO bi POP mS V V V I g 13m ax 1which can be written as ()⎪⎪⎭⎫ ⎝⎛-=PO bi O mS V V G g 1m ax 1 We found 69.21=O G mS, 34.1=bi V V, 69.1=PO V V Then ()()⎪⎪⎭⎫ ⎝⎛-=69.134.1169.2m ax mS g or ()295.0max =mS g mS This is for a channel length of μ10=L m.(b) If the channel length is reduced toμ2=L m, then ()()47.12102947.0max =⎪⎭⎫ ⎝⎛=mS g mS_______________________________________ 13.16n-channel MESFET - GaAs(a) sdPO N ea V ∈=22()()()()()141624191085.81.132105.1105.0106.1---⨯⨯⨯⨯= or 59.2=PO V VNow n Bn bi V φφ-=where ()⎪⎪⎭⎫ ⎝⎛⨯⨯=⎪⎪⎭⎫ ⎝⎛=1617105.1107.4ln 0259.0ln d c t n N N V φ or 0892.0=nφVso that811.00892.090.0=-=bi V V Then59.2811.0-=-=PO bi T V V V or78.1-=T V V(b )If 0<T V for an n-channel device, the device is a depletion mode MESFET._______________________________________13.17 n-channel MESFET - GaAs (a) We want 10.0+=T V VThen PO n Bn PO bi T V V V V --=-=φφsos dd c t T N ea N N V V ∈-⎪⎪⎭⎫ ⎝⎛-==2ln 89.010.02which can be written as ()⎪⎪⎭⎫ ⎝⎛⨯d N 17107.4ln 0259.0 ()()()()10.089.01085.81.1321035.0106.1142419-=⨯⨯⨯+---dNor()⎪⎪⎭⎫ ⎝⎛⨯d N17107.4ln 0259.0 ()79.010453.817=⨯+-d NBy trial and error,15101.8⨯=d N cm 3- (b) At 400=T K()()54.13004003004002/3=⎪⎭⎫⎝⎛=c c N NThen()()()54.1107.440017⨯=c N171024.7⨯=cm 3- Also()03453.03004000259.0=⎪⎭⎫⎝⎛=t VThen()⎪⎪⎭⎫ ⎝⎛⨯⨯-=1517101.81024.7ln 03453.089.0T V ()()1517101.810453.8⨯⨯-- which becomes 050.0+=T V V_______________________________________13.18(a) 2/12⎥⎦⎤⎢⎣⎡∈=d pO s eN V a()()()()()2/1161914102106.15.11085.81.132⎥⎦⎤⎢⎣⎡⨯⨯⨯=- 51030.3-⨯=cm μ330.0=m(b) pO bi T V V V -= We find ()⎪⎪⎭⎫⎝⎛⨯⨯=⎪⎪⎭⎫ ⎝⎛=1617102107.4ln 0259.0ln d c t n N N V φ 0818.0=V 788.00818.087.0=-=-=n Bn bi V φφV Then 712.05.1788.0-=-=T V V(c) ()2/122⎥⎦⎤⎢⎣⎡-+∈=d GS DS bi s eN V V V h ()()()()()2/1161914102106.11085.81.132⎥⎥⎦⎤⎢⎢⎣⎡⨯⨯-+⨯=--GS DS bi V V V (i) ()()[]2/11024.00788.010246.7-+⨯=-h510677.1-⨯=cm μ1677.0=m μ1623.01677.0330.02=-=-h a m (ii)()()[]2/11024.00.1788.010246.7-+⨯=-h510171.3-⨯=cm μ3171.0=m μ0129.03171.0330.02=-=-h a m (iii)()()[]2/11024.00.4788.010246.7-+⨯=-h51064.5-⨯=cm μ564.0=m022=-⇒>h a a h_______________________________________13.19(a) sdpO N ea V ∈=22()()()()()141524191085.81.1321051050.0106.1---⨯⨯⨯⨯=8626.0=V We find()⎪⎪⎭⎫⎝⎛⨯⨯=1517105107.4ln 0259.0n φ1177.0=V1177.087.0-=-=n Bn bi V φφ 7523.0=V8626.07523.0-=-=pO bi T V V V 1103.0-=V(b) ()0713.0103107.4ln 0259.01617=⎪⎪⎭⎫⎝⎛⨯⨯=n φV0713.087.0-=-=n Bn bi V φφ7987.0=VpO bi T V V V -=or ()1103.07987.0--=-=T bi pO V V V 909.0=VThen 2/12⎥⎦⎤⎢⎣⎡∈=d pO s eN V a ()()()()()2/1161914103106.1909.01085.81.132⎥⎦⎤⎢⎣⎡⨯⨯⨯=-- 510095.2-⨯=cm μ2095.0=m_______________________________________13.20PO n Bn PO bi T V V V V --=-=φφ We want 5.0=T V V, so PO n V --=φ85.05.0 Now()⎪⎪⎭⎫ ⎝⎛⨯=d n N17107.4ln 0259.0φ andsdPO N ea V ∈=22()()()()1424191085.81.1321025.0106.1---⨯⨯⨯=dNor()d PO N V 171031.4-⨯= Then()⎪⎪⎭⎫⎝⎛⨯-=d N17107.4ln 0259.085.05.0 ()d N 171031.4-⨯-By trial and error151045.5⨯=d N cm 3-_______________________________________13.21n-channel MESFET - silicon(a) For a gold contact, 82.0=Bn φV. We find()206.010108.2ln 0259.01619=⎪⎪⎭⎫⎝⎛⨯=n φVand 614.0206.082.0=-=-=n Bn bi V φφV With 0=DS V and 35.0=GS V V, we find410075.0-⨯=-h a ()2/12⎥⎦⎤⎢⎣⎡-∈-=d GS bi s eN V V aso that 410075.0-⨯=a()()()()()2/116191410106.135.0614.01085.87.112⎥⎦⎤⎢⎣⎡⨯-⨯+-- or 41026.0-⨯=a cm μ26.0=m Nows dPO bi T N ea V V V ∈-=-=2614.02 or ()()()()()141624191085.87.112101026.0106.1614.0---⨯⨯⨯-=T VWe obtain092.0=T V V (b)()()GS bi PO DS V V V sat V --=()()GS bi T bi V V V V ---= or()092.035.0-=-=T GS DS V V sat V which yields()258.0=sat V DS V_______________________________________13.22(a) ()⎪⎪⎭⎫⎝⎛⨯⨯=1617102107.4ln 0259.0n φ0818.0=V(i) 0818.090.0-=-=n Bn bi V φφ 818.0=V(ii) sdpO N ea V ∈=22()()()()()141624191085.81.1321021065.0106.1---⨯⨯⨯⨯=83.5=V(iii) 83.5818.0-=-=pO bi T V V V 012.5-=V(b) ()()GS bi pO DS V V V sat V --=(i) ()()()0.1818.083.5---=sat V DS01.4=V (ii) ()()()0.2818.083.5---=sat V DS 01.3=V(iii) ()()()0.3818.083.5---=sat V DS01.2=V_______________________________________13.23 (a) aLWk s n n 2∈=μ ()()()()()()44414105.11025.021*******.81.136500----⨯⨯⨯⨯=310206.1-⨯=A/V 2206.1=mA/V 2(b) ()()21T GS n D V V k sat I -=(i) ()()()2115.025.0206.1-=sat I D 01206.0=mA μ06.12= A(ii) ()()()2115.045.0206.1-=sat I D1085.0=mA (c) ()T GS DS V V sat V -=(i) ()10.015.025.0=-=sat V DS V(ii) ()30.015.045.0=-=sat V DS V _______________________________________13.24(a) ()[]2T GS n GSGS D ms V V k V V I g -∂∂=∂∂=()T GS n V V k -=2 ()15.045.0225.1-=n k 083.2=⇒n k mA/V 2 aLWk s n n 2∈=μ()()()()()44143105.11025.021085.81.13650010083.2----⨯⨯⨯=⨯W310073.2-⨯=⇒W cm μ73.20=m (b) ()()21T GS n D V V k sat I -= (i) ()()()2115.025.0083.2-=sat I D 02083.0=mA μ83.20= A(ii) ()()()2115.045.0083.2-=sat I D1875.0=mA_______________________________________13.25 Plot_______________________________________13.26Plot _______________________________________13.27()()()()⎥⎥⎦⎤⎢⎢⎣⎡⨯⨯=2101618105.110310ln 0259.0bi V8424.0=Vsd pO N ea V ∈=22 ()()()()()141624191085.87.1121031050.0106.1---⨯⨯⨯⨯=795.5=V (a) ()()GS bi pO DS V V V sat V --= 953.48424.0795.5=-=V()()2/12⎥⎦⎤⎢⎣⎡-∈=∆d DS DS s eN sat V V L()()()()()2/1161914103106.1953.4101085.87.112⎥⎦⎤⎢⎣⎡⨯⨯-⨯=-- 510666.4-⨯=∆L cm Now()90.0211=∆-='LL L L ()()10.0210666.410.025-⨯=∆=L L410333.2-⨯=L cm μ333.2=m (b) ()()GS bi pO DS V V V sat V --= ()38424.0795.5+-=953.1=V()()()()()2/1161914103106.1953.1101085.87.112⎥⎦⎤⎢⎣⎡⨯⨯-⨯=∆--L510892.5-⨯=cm Then()()10.0210892.510.025-⨯=∆=L L410946.2-⨯=cm μ946.2=m_______________________________________13.28 We have that ()⎪⎪⎭⎫ ⎝⎛∆-='L L LI I D D 2111 Assuming that we are in the saturation region,then ()sat I I D D11'='and ()sat I I D D 11=. We can write()()L L sat I sat I D D ∆⋅-⋅='211111 If L L <<∆, then()()⎥⎦⎤⎢⎣⎡∆⋅+≅'L L sat I sat I D D 21111 We have that()()2/12⎥⎦⎤⎢⎣⎡-∈=∆d DS DS s eN sat V V L()2/112⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛-∈=DS DS d DS s V sat V eN V which can be written as ()2/112⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛-∈=∆DS DS DS d s DS V sat V V eN V L If we write()()()DS D DV sat I sat I λ+='111 then by comparing equations, we have()2/11221⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛-∈=DS DS DSd sV sat V V eN L λ The parameter λis not independent of DS V . Define()sat V V x DS DS≡and consider the function⎪⎭⎫⎝⎛-=x x f 111So that λ is nearly a constant._______________________________________ 13.29 (a) Saturation occurs when 4101⨯=E V/cm.As a first approximation, let L V DS =EThen ()()21021044=⨯=⋅E =-L V DS V (b) We have that ()2/122⎥⎦⎤⎢⎣⎡-+∈==d GS DS bi s sat eN V V V h h and()()()()⎥⎥⎦⎤⎢⎢⎣⎡⨯⨯⨯=2101618105.1104105ln 0259.0bi V or8915.0=bi V V For 0=GS V , we obtain()()()()()2/1161914104106.128915.01085.87.112⎥⎦⎤⎢⎣⎡⨯⨯+⨯=--sat h or410306.0-⨯=sat h cm μ306.0=m(c) We then find ()()W h a eN sat I sat sat d D -=υ1 ()()()7161910104106.1⨯⨯=-()()()44103010306.050.0--⨯-⨯or()72.31=sat I D mA (d) For 0=GS V , we have ()⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛-⎪⎪⎭⎫ ⎝⎛-=PO bi PO biP D VV V V I sat I 3213111 Now()LW a eN I s d n P ∈=6321μ()()()[]()()14216191085.87.116104106.11000--⨯⨯⨯=()()()4344102105.01030---⨯⨯⨯⨯or36.121=P I mAAlso sdPO N ea V ∈=22 ()()()()()141624191085.87.112104105.0106.1---⨯⨯⨯⨯= or 726.7=PO V V Then ()()⎢⎣⎡⎪⎭⎫ ⎝⎛-=726.78915.03136.121sat I D⎥⎥⎦⎤⎪⎪⎭⎫ ⎝⎛-⨯726.78915.0321 or()05.91=sat I D mA_______________________________________13.30(a) If μ1=L m, then saturation will occur when()()11011044=⨯=⋅E =-L V DS V We find()2/122⎥⎦⎤⎢⎣⎡-+∈==d GS DS bi s sat eN V V V h hWe have 8915.0=bi V V and for 0=GS V , we obtain()()()()()2/1161914104106.118915.01085.87.112⎥⎦⎤⎢⎣⎡⨯⨯+⨯=--sathor410247.0-⨯=sat h cm μ247.0=m Then()()W h a eN sat I sat sat d D -=υ1()()()7161910104106.1⨯⨯=-()()()44103010247.050.0--⨯-⨯or()86.41=sat I D mAIf velocity saturation did not occur, then from the previous problem, we would have()()1.181205.91=⎪⎭⎫⎝⎛=sat I D mA(b) If velocity saturation occurs, then the relation ()()L sat I D 11∝does not apply._______________________________________13.31 (a)()()731041058000⨯=⨯=E =n μυcm/s Then 1274105104102--⨯=⨯⨯==υL t d s or 5=d t ps(b) Assume 710==sat υυcm/s Then117410210102--⨯=⨯==satd Lt υs or20=d t ps_______________________________________13.32 (a)()()7410101000==E =n μυcm/s Then117410210102--⨯=⨯==υL t d s or20=d t ps(b) For 710==sat υυcm/s 117410210102--⨯=⨯==satd Lt υs or20=d t ps_______________________________________13.33The reverse-bias current is dominated by the generation current. We have PO bi P V V V -= We find()()()()⎥⎥⎦⎤⎢⎢⎣⎡⨯⨯⨯=2101618105.1103105ln 0259.0bi Vor884.0=bi V VAlso sd PON ea V ∈=22 ()()()()()⎥⎥⎦⎤⎢⎢⎣⎡⨯⨯⨯⨯=---141624191085.87.112103103.0106.1or 086.2=PO V V Then 20.1086.2884.0-=-=P V VLet 20.1-=GS V VNow()2/12⎥⎦⎤⎢⎣⎡-+∈=d GS DS bi s n eN V V V x ()()()⎢⎣⎡⨯⨯=--1914106.11085.87.112 ()()()2/11610320.1884.0⎥⎦⎤⨯--+⨯DS Vor()()[]2/110084.210314.4DS n V x +⨯=- (a) For 0=DS V , μ30.0=n x m (b) For 1=DS V V, μ365.0=n x m (c) For 5=DS V V, μ553.0=n x mThe depletion region volume at the drain is()()()()()W a x W L a Vol n 22+⎪⎭⎫⎝⎛=()()44410302104.2103.0---⨯⎪⎪⎭⎫⎝⎛⨯⨯= ()()()441030106.0--⨯⨯+n xor()8121018108.10--⨯+⨯=n x Vol(a) For 0=DS V , 111062.1-⨯=Vol cm 3 (b) For 1=DS V V, 1110737.1-⨯=Vol cm 3 (c) For 5=DS V V, 1110075.2-⨯=Vol cm 3The generation current at the drain isVol n e I O i DG ⋅⎪⎪⎭⎫⎝⎛=τ2 ()()Vol ⋅⎥⎦⎤⎢⎣⎡⨯⨯⨯=--810191052105.1106.1 or()Vol I DG ⋅⨯=-2104.2(a) For 0=DS V , 39.0=DG I pA(b) For 1=DS V V, 42.0=DG I pA (c) For 5=DS V V, 50.0=DG I pA_______________________________________13.34 (a) The ideal transconductance for 0=GS V is ⎪⎪⎭⎫ ⎝⎛-=PO bi O mS V V G g 11 where L W a N e G d n O μ=1 ()()()41619105.11074500106.1--⨯⨯⨯= ()()44103.0105--⨯⨯⨯ or04.51=O G mS We findsdPO N ea V ∈=22()()()()()141624191085.81.132107103.0106.1---⨯⨯⨯⨯=or347.4=PO V V We have()049.0107107.4ln 0259.01617=⎪⎪⎭⎫⎝⎛⨯⨯=n φVso that841.0049.089.0=-=-=n Bn bi V φφV Then()⎪⎪⎭⎫ ⎝⎛-=347.4841.0104.5mS g or82.2=mS g mS(b) With a source resistancesm m m s m m m r g g g r g g g +='⇒+='111For()sm m r g g 823.21180.0+=='we obtain Ω=6.88s r (c)A L A L r s σρ==()An e L n μ=so ()()()()16191074500106.156.88⨯⨯=-L()()44105103.0--⨯⨯⨯ or41067.0-⨯=L cm μ67.0=m _______________________________________13.35Considering the capacitance charging time,we haveG m T C g f π2= where a W L C s G ∈= ()()()()44414103.0105.11051085.81.13----⨯⨯⨯⨯=or 15109.2-⨯=G C F We must use mg ', so we obtain ()()()153109.2280.01082.2--⨯⨯=πT f 1110238.1⨯= Hz We can also write TC C T f f πττπ2121=⇒= so()121110285.110238.121-⨯=⨯=πτC s The channel transit time is 1174105.110105.1--⨯=⨯=t t s The total time constant is121110285.1105.1--⨯+⨯=τ 1110629.1-⨯= s Taking into account the channel transit time and the capacitance charging time, we find()1110629.12121-⨯==πτπT f or 91077.9⨯=T f Hz 77.9=GHz _______________________________________13.36(a) For constant mobility 222L a N e f s d n T ∈=πμ ()()()()()()()2414241619102.11085.81.1321030.010********.1----⨯⨯⨯⨯⨯=π111012.4⨯=T f Hz 412=GHz (b) For saturation velocity model ()47102.12102-⨯==ππυL f sat T101033.1⨯=T f Hz 3.13=GHz_______________________________________13.37 222L a N e f s d n T ∈=πμ()()()()()()2142416191085.87.1121040.010********.1L ---⨯⨯⨯⨯=π 2975.786L f T = (a) ()24103975.786-⨯=T f 91074.8⨯=Hz 74.8=GHz (b) ()24105.1975.786-⨯=T f 101050.3⨯=Hz 0.35=GHz_______________________________________ 13.38 222L a N e f s a p T ∈=πμ or2/122⎥⎥⎦⎤⎢⎢⎣⎡∈=T s a p f a N e L πμ ()()()()()()2/1142416191085.87.1121040.010*******.1⎥⎥⎦⎤⎢⎢⎣⎡⨯⨯⨯⨯=---T f π T f L 18.18=(a) 910518.18⨯=L 41057.2-⨯=cm μ57.2=m (b) 9101218.18⨯=L41066.1-⨯=cm μ66.1=m_______________________________________13.39 (a)2P cB off V eE V -∆-=φwhereNdd P d eN V ∈=222()()()()()142818191085.82.12210350103106.1---⨯⨯⨯⨯=or72.22=P V V Then72.224.089.0--=off V or07.2-=off V V (b)()()off g NS V V d d e n -∆+∈=For 0=g V , we have()()()()()()07.21080350106.11085.82.1281914---+⨯⨯=Sn or121025.3⨯=S n cm 2-_______________________________________13.40(a) We have()()()sO off g N D V V V d d Wsat I υ⋅--∆+∈=We find()()d d W sat I V W g sN D g mS ∆+∈=⎥⎦⎤⎢⎣⎡∂∂=⎪⎪⎭⎫ ⎝⎛υ()()()()()871410803501021085.82.12--+⨯⨯=or02.5=⎪⎪⎭⎫⎝⎛W g mS S/cm 502=mS/mm(b) At 0=g V , we obtain ()()()s O off N D V V d d Wsat I υ⋅--∆+∈= ()()()()()()7812102107.210803501085.82.12⨯-+⨯=-- or()37.5=Wsat I D A/cm 537=mA/mm _______________________________________13.412P cB off V eE V -∆-=φWe want 3.0-=off V V, so 222.085.030.0P V --=- or93.02=P V V We haveNdd P d eN V ∈=222ordP N d eN V d 222∈=()()()()()181914102106.193.01085.82.122⨯⨯⨯=-- We then obtain61051.2-⨯=d d cm oA 251=_______________________________________。

半导体物理与器件第四版课后习题答案9

______________________________________________________________________________________Chapter 99.1(a) We have⎪⎪⎭⎫⎝⎛=d c t n N N eV e ln φ()206.010108.2ln 0259.01619=⎪⎪⎭⎫⎝⎛⨯=eV(c)01.428.4-=-=χφφm BO or27.0=BO φV and206.027.0-=-=n BO bi V φφ or064.0=bi V V Also2/12⎥⎦⎤⎢⎣⎡∈=d bi s d eN V x()()()()()2/116191410106.1064.01085.87.112⎥⎦⎤⎢⎣⎡⨯⨯=-- or6101.9-⨯=d x cm Thensdd x eN ∈=E max()()()()()14616191085.87.1110`1.910106.1---⨯⨯⨯=or4max 1041.1⨯=E V/cm (d)Using the figure, 55.0=Bn φV So206.055.0-=-=n Bn bi V φφ or344.0=bi V V We then find51011.2-⨯=n x cm and4max 1026.3⨯=E V/cm_______________________________________ 9.2(a)n B bi V φφ-=0 ⎪⎪⎭⎫ ⎝⎛=d ct n NN V ln φ ()⎪⎪⎭⎫⎝⎛⨯⨯=1519105108.2ln 0259.0=0.2235 V4265.02235.065.0=-=bi V V (b) ()⎪⎪⎭⎫⎝⎛⨯=161910108.2ln 0259.0n φ2056.0=V4444.02056.065.0=-=bi V V bi V increases, 0B φremains constant (c) ()⎪⎪⎭⎫⎝⎛⨯=151910108.2ln 0259.0n φ2652.0=V3848.02652.065.0=-=bi V V bi V decreases, 0B φremains constant_______________________________________ 9.3(a)09.101.41.50=-=-=χφφm B V (b)n B bi V φφ-=0 ⎪⎪⎭⎫⎝⎛=d c t n N N V ln φ()2056.010108.2ln 0259.01619=⎪⎪⎭⎫⎝⎛⨯=V8844.02056.009.1=-=bi V V (c) ()2/12⎥⎦⎤⎢⎣⎡+∈=d R bi s n eN V V x(i)()()()()()2/116191410106.118844.01085.87.112⎥⎦⎤⎢⎣⎡⨯+⨯=--n x510939.4-⨯=cm______________________________________________________________________________________or μ4939.0=n x msnd x eN ∈=E max()()()()()14516191085.87.1110939.410106.1---⨯⨯⨯= 41063.7⨯=V/cm(ii)()()()()()2/116191410106.158844.01085.87.112⎥⎦⎤⎢⎣⎡⨯+⨯=--n x 510727.8-⨯=cm or μ8728.0=n x m()()()()()1451619max 1085.87.1110727.810106.1---⨯⨯⨯=E51035.1⨯=V/cm_______________________________________ 9.4(a)03.107.41.50=-=-=χφφm B V (b) ()1177.0105107.4ln 0259.01517=⎪⎪⎭⎫⎝⎛⨯⨯=n φV(c)9123.01177.003.1=-=bi V V (d)(i)()()()()()2/1151914105106.119123.01085.81.132⎥⎦⎤⎢⎣⎡⨯⨯+⨯=--n x 510445.7-⨯=cm or μ7445.0=n x m()()()()()1451519max 1085.81.1310445.7105106.1---⨯⨯⨯⨯=E41014.5⨯=V/cm(ii)()()()()()2/1151914105106.159123.01085.81.132⎥⎦⎤⎢⎣⎡⨯⨯+⨯=--n x 410309.1-⨯=cm or μ309.1=n x m()()()()()1441519max 1085.81.1310309.1105106.1---⨯⨯⨯⨯=E41003.9⨯=V/cm_______________________________________ 9.5(b) 1177.0=n φV(c) 7623.01177.088.0=-=bi V V(d) (i)()()()()()2/1151914105106.117623.01085.81.132⎥⎦⎤⎢⎣⎡⨯⨯+⨯=--n x 510147.7-⨯=cm or μ7147.0=n x m()()()()()1451519max 1085.81.1310147.7105106.1---⨯⨯⨯⨯=E 41093.4⨯=V/cm (ii)()()()()()2/1151914105106.157623.01085.81.132⎥⎦⎤⎢⎣⎡⨯⨯+⨯=--n x 410292.1-⨯=cm or μ292.1=n x m()()()()()1441519max 1085.81.1310292.1105106.1---⨯⨯⨯⨯=E41092.8⨯=V/cm_______________________________________ 9.6(a) ()2/12⎥⎦⎤⎢⎣⎡+∈='R bid s V V Ne CWe have 88.00=B φV()⎪⎪⎭⎫⎝⎛⨯=151910108.2ln 0259.0n φ265.0=V615.0265.088.0=-=bi V V (i)()()()()()()2/115141941615.02101085.87.11106.110⎥⎦⎤⎢⎣⎡+⨯⨯=---C 131016.7-⨯= F or 716.0=C pF (ii)______________________________________________________________________________________()()()()()()2/115141945615.02101085.87.11106.110⎥⎦⎤⎢⎣⎡+⨯⨯=---C 131084.3-⨯= F or 384.0=C pF (b) ()206.010108.2ln 0259.01619=⎪⎪⎭⎫⎝⎛⨯=n φV 674.0206.088.0=-=bi V V (i) ()()()()()()2/116141941674.02101085.87.11106.110⎥⎦⎤⎢⎣⎡+⨯⨯=---C 121022.2-⨯= F or 22.2=C pF (ii) ()()()()()()2/1161419456745.02101085.87.11106.110⎥⎦⎤⎢⎣⎡+⨯⨯=---C 121021.1-⨯= F or 21.1=C pF_______________________________________ 9.7 (a) From the figure, 90.0=bi V V(b) We find()1515210034.190.0201031⨯=---⨯=∆⎪⎭⎫ ⎝⎛'∆R V C and d s N e ∈=⨯210034.115 We can then write ()()()()15141910034.11085.81.13106.12⨯⨯⨯=--d N or 161004.1⨯=d N cm 3-(c)⎪⎪⎭⎫⎝⎛=d c t n N N V ln φ ()⎪⎪⎭⎫⎝⎛⨯⨯=16171004.1107.4ln 0259.0or0986.0=n φV (d)0986.090.0+=+=n bi Bn V φφ or9986.0=Bn φV_______________________________________9.8 From Figure 9.5, 63.0≅BO φV(a) ()224.0105108.2ln 0259.01519=⎪⎪⎭⎫ ⎝⎛⨯⨯=n φV406.0224.063.00=-=-=n B bi V φφV(i)()()()()()2/1151914105106.11406.01085.87.112⎥⎦⎤⎢⎣⎡⨯⨯+⨯=--n x 510033.6-⨯=cmor μ6033.0=n x m ()()()()()1451519max 1085.87.1110033.6105106.1---⨯⨯⨯⨯=E 41066.4⨯=V/cm (ii)()()()()()2/1151914105106.15406.01085.87.112⎥⎦⎤⎢⎣⎡⨯⨯+⨯=--n x 410183.1-⨯=cmor μ183.1=n x m ()()()()()1441519max 1085.87.1110183.1105106.1---⨯⨯⨯⨯=E41014.9⨯=V/cm (b)(i) se ∈E=∆πφ4 ()()()()2/1144191085.87.1141066.4106.1⎥⎦⎤⎢⎣⎡⨯⨯⨯=--π 0239.0=V E ∈=s m e x π16()()()()2/1414191066.41085.87.1116106.1⎥⎦⎤⎢⎣⎡⨯⨯⨯=--πor______________________________________________________________________________________ m x 71057.2-⨯=cm (ii) ()()()()2/1144191085.87.1141014.9106.1⎥⎦⎤⎢⎣⎡⨯⨯⨯=∆--πφ 0335.0=V()()()2/1414191014.91085.87.1116106.1⎥⎦⎤⎢⎣⎡⨯⨯⨯=--πm x 71083.1-⨯=cm_______________________________________ 9.9 We have ()x x ex s E -∈-=-πφ16 or()ex xe x e s E +∈=πφ162 Now ()()e x e dx x e d s E +∈-==22160πφ Solving for x , we find E∈==s m ex x π16Substituting this value of m x x = into the equation for the potential, we find E∈E+E∈∈=∆s s see e πππφ161616which yields se ∈E=∆πφ4 _______________________________________ 9.10From Figure 9.5, 88.0≅BO φV(a) ()0997.010107.4ln 0259.01617=⎪⎪⎭⎫⎝⎛⨯=n φV780.00997.088.00≅-=-=n B bi V φφV()()()()()2/116191410106.1780.01085.81.132⎥⎦⎤⎢⎣⎡⨯⨯=--n x510362.3-⨯=cmor μ3362.0=n x m()()()()()1451619max 1085.81.1310362.310106.1---⨯⨯⨯=E 41064.4⨯=V/cm (b) ()()044.088.005.0==∆φV s e ∈E =π4()()()()141921085.81.134106.1044.0--⨯E ⨯=π ()()()()19142106.11085.81.134044.0--⨯⨯=E π510763.1⨯=V/cmNow ()()()()14161951085.81.1310106.110763.1--⨯⨯=⨯=E n x 410277.1-⨯=⇒n x cmAnd ()24210277.1-⨯=n x()()()()()16191410106.1780.01085.81.132--⨯+⨯=R V5.10=⇒R V V_______________________________________ 9.11Plot_______________________________________ 9.12(a) 07.42.5-=-=χφφm BO or13.1=BO φV(b) We have()Bn O g e e E φφ--()n Bn d s itN e eD φφ-∈=21()[]Bn m it ieD φχφδ+-∈-which becomes()Bn e φ--60.043.1______________________________________________________________________________________ ()()()[1419131085.81.13106.12101--⨯⨯⎪⎪⎭⎫ ⎝⎛=e e()()]2/11610.010-⨯Bn φ()()()[]Bn e e φ+-⨯⎪⎪⎭⎫ ⎝⎛⨯---07.42.51025101085.881314orBn φ-83.0()Bn Bn φφ---=13.1221.010.0038.0 We find858.0=Bn φV (c)If 5.4=m φV, then07.45.4-=-=χφφm BO or43.0=BO φVFrom part (b), we have Bn φ-83.0()[]Bn Bn φφ+---=07.45.4221.010.0038.0 We then find733.0=Bn φVWith interface states, the barrier height is lesssensitive to the metal work function._______________________________________ 9.13We have that()Bn O g e e E φφ--()n Bn d s itN e eD φφ-∈=21()[]Bn m it ieD φχφδ+-∈-Let itit D eD '=(cm 2-eV 1-) Then we can write()60.0230.012.1--e()()()[14191085.87.11106.121--⨯⨯'=itD ()()]2/116164.060.0105-⨯⨯ ()()()[]60.001.475.410201085.8814+-⨯'⨯---itD We then find111097.4⨯='itD cm 2-eV 1- _______________________________________ 9.14(a) ()224.0105108.2ln 0259.01519=⎪⎪⎭⎫⎝⎛⨯⨯=n φV(b)666.0224.089.0=-=-=n Bn bi V φφV (c)⎪⎪⎭⎫⎝⎛-=*kT e T A J Bn sT φexp 2()()⎪⎭⎫ ⎝⎛-=0259.089.0exp 300120281029.1-⨯=sT J A/cm 2 (d)()⎪⎭⎫ ⎝⎛⨯=⎪⎪⎭⎫ ⎝⎛=-81029.15ln 0259.0ln sT t a J J V V 512.0=a V V_______________________________________ 9.15(a)63.00≅B φV ()()⎪⎭⎫ ⎝⎛-=0259.063.0exp 3001202sT J410948.2-⨯=A/cm 2()()84410948.210948.210---⨯=⨯=sT I A(i) ⎪⎪⎭⎫ ⎝⎛=sT t a IIV V ln ()⎪⎪⎭⎫ ⎝⎛⨯⨯=--8610948.21010ln 0259.0 151.0=V______________________________________________________________________________________(ii) ()⎪⎪⎭⎫⎝⎛⨯⨯=--8610948.210100ln 0259.0a V 211.0=V (iii) ()⎪⎪⎭⎫ ⎝⎛⨯=--8310948.210ln 0259.0a V 270.0=V (b) ()030217.03003500259.0=⎪⎭⎫ ⎝⎛=kT eV ()()()⎪⎭⎫ ⎝⎛-=-030217.063.0exp 3501201024sT I610296.1-⨯= A (i) ⎥⎥⎦⎤⎢⎢⎣⎡-⎪⎪⎭⎫ ⎝⎛=1exp t a sT V V I I ()⎥⎥⎦⎤⎢⎢⎣⎡+⨯⨯=--110296.11010ln 030217.066a V 0654.0=V (ii) ()⎥⎦⎤⎢⎣⎡+⨯⨯=--110296.110100ln 030217.066a V 1317.0=V(iii) ()⎪⎪⎭⎫ ⎝⎛⨯≅--6310296.110ln 030217.0a V 201.0=V _______________________________________9.16(a) 88.0≅Bn φV (b) ()()⎪⎭⎫ ⎝⎛-=0259.088.0exp 30012.12sT J1010768.1-⨯=A/cm 2(c) ()⎪⎭⎫ ⎝⎛⨯=-1010768.110ln 0259.0a V 641.0=V(d) ()()()2ln 0259.02ln ==∆t a V V 0180.0=V_______________________________________9.17Plot _______________________________________9.18From the figure, 68.0=Bn φV ⎪⎪⎭⎫ ⎝⎛∆⋅⎪⎪⎭⎫ ⎝⎛-=t t Bn ST V V T A J φφexp exp 2* ()()⎪⎪⎭⎫ ⎝⎛∆⋅⎪⎭⎫ ⎝⎛-=t V φexp 0259.068.0exp 3001202 or⎪⎪⎭⎫ ⎝⎛∆⨯=-t ST V J φexp 10277.45We have s e ∈E =∆πφ4 Now ⎪⎪⎭⎫⎝⎛=d c t n N N V ln φ()2056.010108.2ln 0259.01619=⎪⎪⎭⎫⎝⎛⨯=Vand 4744.02056.068.0=-=-=n Bn bi V φφV (a) We find for 2=R V V, ()2/12⎥⎦⎤⎢⎣⎡+∈=d R bi s d eN V V x()()()()()2/116191410106.14744.21085.87.112⎥⎦⎤⎢⎣⎡⨯⨯=-- or 410566.0-⨯=d x cm μ566.0=m Then s dd x eN ∈=E max ()()()()()14416191085.87.1110566.010106.1---⨯⨯⨯= or 4max 10745.8⨯=E V/cm Now()()()()2/1144191085.87.11410745.8106.1⎥⎦⎤⎢⎣⎡⨯⨯⨯=∆--πφ______________________________________________________________________________________ or 0328.0=∆φV Then()⎪⎭⎫ ⎝⎛⨯=-0259.00328.0exp 10277.451ST J or 411052.1-⨯=ST J A/cm 2 For 410-=A cm 2, we find 811052.1-⨯=R I A (b) For 4=R V V, then()()()()()2/116191410106.14744.41085.87.112⎥⎦⎤⎢⎣⎡⨯⨯=--d x or410761.0-⨯=d x cm μ761.0=mAlso()()()()()1441619max 1085.87.1110761.010106.1---⨯⨯⨯=Eor5max 10176.1⨯=E V/cm and()()()()2/1145191085.87.11410176.1106.1⎥⎦⎤⎢⎣⎡⨯⨯⨯=∆--πφ or03803.0=∆φV Then()⎪⎭⎫⎝⎛⨯=-0259.003803.0exp 10277.452ST Jor421086.1-⨯=ST J A/cm 2 Finally,821086.1-⨯=R I A_______________________________________ 9.19We have thatdn JcE x m s ⎰∞-→=υThe incremental electron concentration is ()()dE E f E g dn F c = where()()c nc E E hm E g -=32/3*24π and assuming the Boltzmannapproximation ()()⎥⎦⎤⎢⎣⎡--=kT E E E f F F exp Then()c nE E h m dn -=32/3*24π()dE kT E E F ⎥⎦⎤⎢⎣⎡--⨯exp If the energy above c E is kinetic energy, thenc n E E m -=2*21υWe can then write2*nc m E E υ=-andυυυυd m d m dE n n **221=⋅=We can also write()()F c c F E E E E E E -+-=-n n e m φυ+=2*21 so that⎪⎪⎭⎫⎝⎛-⎪⎪⎭⎫ ⎝⎛=kT e h m dn n n φexp 23*υυπυd kT m n 22*42exp ⋅⎪⎪⎭⎫ ⎝⎛-⨯We can write2222z y x υυυυ++=The differential volume element is z y x d d d d υυυυυπ=24The current is due to all x-directed velocitiesthat are greater than Ox υ and for all y- andz- directed velocities. Then______________________________________________________________________________________⎪⎪⎭⎫ ⎝⎛-⎪⎪⎭⎫ ⎝⎛=-→kT e h m J n n m s φexp 23*xx n x d kT m Oxυυυυ⎪⎪⎭⎫⎝⎛-⨯⎰∞2exp 2*y y n d kT m υυ⎰∞∞-⎪⎪⎭⎫⎝⎛-⨯2exp 2*z z n d kT m υυ⎰∞∞-⎪⎪⎭⎫ ⎝⎛-⨯2exp 2* We can write ()a bi Ox n V V e m -=2*21υMake a change of variables:()kTV V kT m a bi x n -+=2222*αυ or()⎥⎦⎤⎢⎣⎡-+=kT V V e m kT a bi n x 2*22αυ Taking the differential, we findααυυd m kT d n x x ⎪⎪⎭⎫⎝⎛=*2We may note that when Ox x υυ=, 0=α. We may define other change of variables,βυβυ⋅⎪⎪⎭⎫⎝⎛=⇒=2/1*22*22n y yn m kT kT mγυγυ⋅⎪⎪⎭⎫ ⎝⎛=⇒=2/1*22*22n z zn m kT kT m Substituting the new variables, we have⎪⎪⎭⎫ ⎝⎛-⋅⎪⎪⎭⎫ ⎝⎛⋅⎪⎪⎭⎫ ⎝⎛=-→kT e m kT h m J n n n m s φexp 222*3*()()αααd kT V V e a bi ⎰∞-⋅⎥⎦⎤⎢⎣⎡--⨯02exp exp ()()γγββd d ⎰⎰∞∞-∞∞--⋅-⨯22exp exp _______________________________________ 9.20 For the Schottky diode, ()()⎪⎪⎭⎫ ⎝⎛⨯=⨯---t a V V exp 106101080.0843 (a) ()()()()⎥⎦⎤⎢⎣⎡⨯⨯=---843106101080.0ln 0259.0SB V a 4845.0=VThen()7695.0285.04845.0=+=pn V a V(b) ()⎪⎭⎫⎝⎛=⨯--0259.07695.0exp 101080.0113pn A 551010998.0--≅⨯=⇒pn A cm 2 _______________________________________9.21 For the pn junction,()()16134104.6108108---⨯=⨯⨯=s I A(a) ()⎪⎪⎭⎫⎝⎛⨯⨯=--166104.610150ln 0259.0a V678.0=V(b) ()⎪⎪⎭⎫⎝⎛⨯⨯=--166104.610700ln 0259.0a V718.0=V(c) ()⎪⎪⎭⎫⎝⎛⨯⨯=--163104.6102.1ln 0259.0a V732.0=VFor the Schottky junction,()()1294108.4106108---⨯=⨯⨯=sT I A(a) ()⎪⎪⎭⎫ ⎝⎛⨯⨯=--126108.410150ln 0259.0a V 447.0=V______________________________________________________________________________________(b) ()⎪⎪⎭⎫⎝⎛⨯⨯=--126108.410700ln 0259.0a V 487.0=V(c) ()⎪⎪⎭⎫⎝⎛⨯⨯=--123108.4102.1ln 0259.0a V 501.0=V_______________________________________9.22 (a) (i) 80.0=I mA in each diode (ii)()()()()⎥⎦⎤⎢⎣⎡⨯⨯⨯=---943106108108.0ln 0259.0SB V a 490.0=V ()()()()⎥⎦⎤⎢⎣⎡⨯⨯⨯=---1343108108108.0ln 0259.0pn V a 721.0=V (b) Same voltage across each diodepn SB I I I +=⨯=-3108.0()()⎪⎪⎭⎫ ⎝⎛⨯⨯=--t aV V exp 10610894()()⎪⎪⎭⎫ ⎝⎛⨯⨯+--t a V V exp 108108134()⎪⎪⎭⎫ ⎝⎛⨯+⨯=--t a V V exp 104.6108.41612Then ()⎥⎦⎤⎢⎣⎡⨯+⨯⨯=---16123104.6108.4108.0ln 0259.0a V 49032.0=a V V ()⎪⎭⎫ ⎝⎛⨯=-0259.049032.0exp 108.412SB I 7998.0=⇒SB I mA ()⎪⎭⎫ ⎝⎛⨯=-0259.049032.0exp 104.616pn Iμ107.0≅⇒pn I A _______________________________________9.23(a) For 8.0=I mA, we find 143.1107108.043=⨯⨯=--J A/cm 2 We have⎪⎪⎭⎫ ⎝⎛=S t a J J V V lnFor the pn junction diode, ()6907.0103143.1ln 0259.012=⎪⎭⎫ ⎝⎛⨯=-a V V For the Schottky diode,()4447.0104143.1ln 0259.08=⎪⎭⎫⎝⎛⨯=-a V V (b) For the pn junction diode,⎪⎪⎭⎫ ⎝⎛-⎪⎭⎫ ⎝⎛∝∝kT E T n J g i S exp 30032Then()()3300400300400⎪⎭⎫⎝⎛=SS J J()()⎥⎦⎤⎢⎣⎡+-⨯0259.03004000259.0exp g g E E⎥⎦⎤⎢⎣⎡-=03453.012.10259.012.1exp 37.2 or()()51017.1300400⨯=S SJ J Now ()()()12541031017.1107--⨯⨯⨯=I⎪⎭⎫ ⎝⎛⨯03453.06907.0exp or120=I mA For the Schottky diode,⎪⎪⎭⎫⎝⎛-∝kT e T J BO ST φexp 2Now ()()2300400300400⎪⎭⎫⎝⎛=ST ST J J ()()⎥⎦⎤⎢⎣⎡+-⨯0259.03004000259.0exp BO BO φφ______________________________________________________________________________________ ⎥⎦⎤⎢⎣⎡-=03453.082.00259.082.0exp 778.1or()()310856.4300400⨯=ST ST J J Then ()()()83410410856.4107--⨯⨯⨯=I⎪⎭⎫ ⎝⎛⨯03453.04447.0expor3.53=I mA_______________________________________9.24 Plot _______________________________________9.25 (a) Ω===--1.0101034A R R c(b) Ω===--1101044A R R c (c) Ω===--10101054A R R c _______________________________________9.26(a) Ω=⨯==--51010555A R R c (i) ()()551===IR V mV(ii) ()()5.051.0===IR V mV (b) 501010565=⨯=--R Ω (i) ()()50501===IR V mV (ii) ()()5501.0===IR V mV _______________________________________ 9.27 2exp T A V V R t Bn t c *⎪⎪⎭⎫⎝⎛=φ or ⎥⎥⎦⎤⎢⎢⎣⎡=*t c t Bn V T A R V 2ln φ (a) ()()()()⎥⎥⎦⎤⎢⎢⎣⎡⨯=-0259.0300120105ln 0259.025Bn φ258.0=V(b) ()()()()⎥⎥⎦⎤⎢⎢⎣⎡⨯=-0259.0300120105ln 0259.026Bn φ 198.0=V_______________________________________ 9.28 (b) We need 20.00.42.4=-=-=χφφm n V And ⎪⎪⎭⎫⎝⎛=d c t n N N V ln φ or ()⎪⎪⎭⎫⎝⎛⨯=d N 19108.2ln 0259.020.0 which yields161024.1⨯=d N cm 3- (c)Barrier height = 0.20 V _______________________________________ 9.29 We have that ()x x eN n s d-∈-=E Then 222C x x x eN dx n s d +⎪⎪⎭⎫ ⎝⎛-⋅∈=E -=⎰φ Let 0=φ at 002=⇒=C x , so⎪⎪⎭⎫⎝⎛-⋅∈=22x x x eN n s d φ At n x x =, bi V =φ, so 22n s d bi x eN V ⋅∈==φ or______________________________________________________________________________________dbis n eN V x ∈=2 Also n BO bi V φφ-= where ⎪⎪⎭⎫ ⎝⎛=d c t n N N V ln φ Now for35.0270.02===BO φφV we have()()()()[8141910501085.87.11106.135.0---⨯⨯⨯=nd x N()⎥⎥⎦⎤⨯--2105028 or ()81410251073.735.0--⨯-⨯=n d x N We have ()()()2/11914106.11085.87.112⎥⎥⎦⎤⎢⎢⎣⎡⨯⨯=--d bi n N V x andn bi V φ-=70.0By trial and error, we find 18105.3⨯=d N cm 3-_______________________________________9.30(b) ⎪⎪⎭⎫ ⎝⎛==a t p BO N N V υφφln ()⎪⎪⎭⎫⎝⎛⨯⨯=16191051004.1ln 0259.0 or 138.0=BO φV_______________________________________9.31 Sketches_______________________________________ 9.32Sketches_______________________________________9.33Electron affinity rule ()p n c e E χχ-=∆ For GaAs, 07.4=χ and for AlAs,5.3=χ. If we assume a linear extrapolation between GaAs and AlAs, then for Al 3.0Ga 7.0As 90.3=⇒χThen 17.090.307.4=-=∆c E eV _______________________________________9.34 Consider an n-P heterojunction in thermal equilibrium. Poisson's equation is()dx d x dx d E-=∈-=ρφ22 In the n-region, ()ndn n n eN x dx d ∈=∈=E ρ For uniform doping, we have1C xeN ndn n +∈=E The boundary condition is0=E n at n x x -=, so we obatinnn dn x eN C ∈=1Then ()n n dnn x x eN +∈=E In the P-region,PaP p eNdx d ∈-=E which gives 2C x eN P aP P +∈-=E We have the boundary condition that 0=E P at P x x =, so that______________________________________________________________________________________PPaP x eN C ∈=2 Then()x x eN P P aP P -∈=E Assuming zero surface charge density at0=x , the electric flux density D iscontinuous, so ()()00P P n n E =∈E ∈,whichyieldsP aP n dn x N x N = We can determine the electric potential as ()dx x n n ⎰E -=φ322C x x eN x eN n n dn n dn +⎥⎥⎦⎤⎢⎢⎣⎡∈+∈-=Now()()n n n bin x V --=φφ0⎥⎥⎦⎤⎢⎢⎣⎡∈+∈--=n ndn n n dn x eN x eN C C 22332ornndn bin x eN V ∈=22Similarly on the P-side, we findPPaP biP x eN V ∈=22We have thatPPaP n n dn biP bin bi x eN x eN V V V ∈+∈=+=2222 We can write⎪⎪⎭⎫⎝⎛=aP dn n P N N x x Substituting and collecting terms, we find222n aP P n dn n aP dn P bi x N N e N N e V ⋅⎥⎥⎦⎤⎢⎢⎣⎡∈∈∈+∈=Solving for n x , we have()2/12⎥⎦⎤⎢⎣⎡∈+∈∈∈=dn n aPP dn bi aP P n n N N eN V N x Similarly on the P-side, we have()2/12⎥⎦⎤⎢⎣⎡∈+∈∈∈=dn n aP P aP bidn P n P N N eN V N xThe total space charge width is then P n x x W += Substituting and collecting terms, we obtain ()()2/12⎥⎦⎤⎢⎣⎡∈+∈+∈∈=dn n aPP aP dn dn aP bi P n N N N eN N N V W _______________________________________。

半导体物理与器件英文版第四版课后练习题含答案

半导体物理与器件英文版第四版课后练习题含答案Chapter 1: Crystal PropertiesMultiple Choice Questions1.Which of the following statements is correct? A. The latticestructure of a crystal can be described by three crystal axes that are normal to each other. B. For a crystal with a primitive cubic unit cell, the coordination number is 8. C. In a crystal lattice with a face-centered cubic (FCC) unit cell, each atom has only six nearest neighbors. D. The Miller indices of a crystal planeperpendicular to the x-axis and passing through point (1, 2, 3) are (1, 2, 3).Answer: A2.Which of the following statements is correct? A. The crystalstructure of diamond is face-centered cubic (FCC). B. The density of silicon is smaller than that of germanium. C. The coordination number of germanium is 4. D. The Miller indices of a crystal plane parallel to the x-axis and passing through point (1, 2, 3) are (1, 0, 0).Answer: DShort Answer Questions1.What is the difference between a lattice and a unit cell?2.Define the concept of coordination number and give anexample of a coordination number 6 crystal structure.3.Define the concept of a crystal plane and expln how Millerindices are used to describe crystal planes.Answers:1.A lattice is an infinitely repeating arrangement of pointsin space that defines the basic symmetry of a crystal, while aunit cell is the smallest repeating unit of a crystal lattice that can be used to reconstruct the entire crystal by translation.2.Coordination number is the number of nearest neighbors of anatom in a crystal lattice. An example of a coordination number 6 crystal structure is the hexagonal close-packed (HCP) structure.3.A crystal plane is an imaginary flat surface in a crystalthat can be used to define the orientation of the crystal in space.Miller indices are a set of integers that describe the orientation of a crystal plane relative to the crystal axes. The Millerindices of a plane are determined by finding the reciprocals of the intercepts of the plane with the crystal axes and thenreducing these reciprocals to the smallest set of integers that give a unique designation of the plane.。

半导体物理与器件第四版课后答案第七章

17
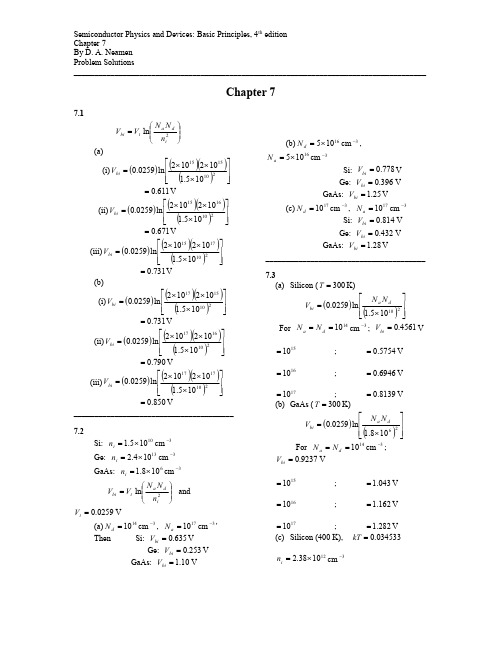
or
x p 0.0213 10 4 cm 0.0213 m
We have
max
19
eN d x n s
15 4

1.6 10 5 10 0.426 10 11.7 8.85 10
14
or
max 3.29 10 4 V/cm

7.4 (a) n-side
Nd E F E Fi kT ln n i

5 1015 1017
1 17 10 5 1015

1/ 2
5 1015 0.0259 ln 1.5 1010 or E F E Fi 0.3294 eV p-side Na E Fi E F kT ln n i 10 0.0259 ln 1.5 1010 or E Fi E F 0.4070 eV (b) Vbi 0.3294 0.4070 or Vbi 0.7364 V (c)
(a) N d 1014 cm 3 , N a 1017 cm 3 ' Si: Vbi 0.635 V Ge: Vbi 0.253 V GaAs: Vbi 1.10 V
; 1017 (c) Silicon (400 K),
n i 2.38 1012 cm 3
Semiconductor Physics and Devices: Basic Principles, 4th edition Chapter 7 By D. A. Neamen Problem Solutions ______________________________________________________________________________________ For N a N d 1014 cm 3 ; Na Nd Vbi Vt ln n2 Vbi 0.2582 V i

半导体物理与器件第四版课后习题答案2

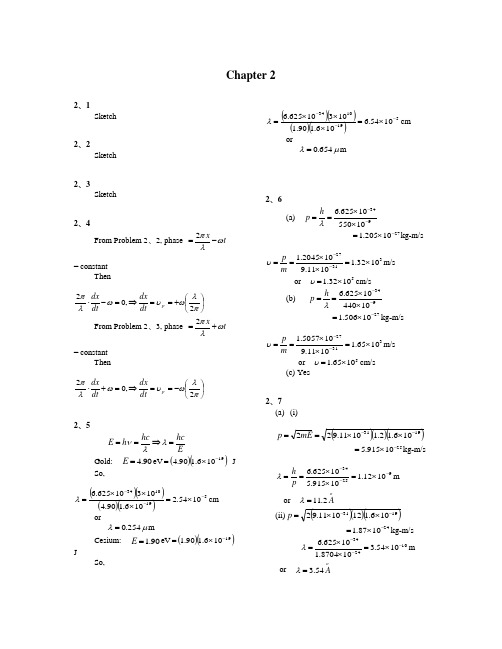
Chapter 22、1Sketch_______________________________________2、2Sketch_______________________________________2、3Sketch_______________________________________2、4From Problem 2、2, phase t xωλπ-=2= constant Then⎪⎭⎫ ⎝⎛+==⇒=-⋅πλωυωλπ2,02p dt dx dt dx From Problem 2、3, phase t xωλπ+=2 = constantThen ⎪⎭⎫⎝⎛-==⇒=+⋅πλωυωλπ2,02p dt dx dt dx _______________________________________2、5E hchc h E =⇒==λλν Gold: 90.4=E eV ()()19106.190.4-⨯= JSo, ()()()()51910341054.2106.190.410310625.6---⨯=⨯⨯⨯=λcm orμλ254.0=m Cesium: 90.1=E eV ()()19106.190.1-⨯= JSo,()()()()51910341054.6106.190.110310625.6---⨯=⨯⨯⨯=λcm orμλ654.0=m_______________________________________2、6(a) 9341055010625.6--⨯⨯==λhp2710205.1-⨯=kg-m/s 331271032.11011.9102045.1⨯=⨯⨯==--m p υm/s or 51032.1⨯=υcm/s(b) 9341044010625.6--⨯⨯==λh p 2710506.1-⨯=kg-m/s331271065.11011.9105057.1⨯=⨯⨯==--m p υm/s or 51065.1⨯=υcm/s (c) Yes_______________________________________ 2、7(a) (i) ()()()1931106.12.11011.922--⨯⨯==mE p 2510915.5-⨯=kg-m/s925341012.110915.510625.6---⨯=⨯⨯==p h λm or o A 2.11=λ(ii)()()()1931106.1121011.92--⨯⨯=p 241087.1-⨯=kg-m/s1024341054.3108704.110625.6---⨯=⨯⨯=λm or oA 54.3=λ(iii) ()()()1931106.11201011.92--⨯⨯=p 2410915.5-⨯=kg-m/s1024341012.110915.510625.6---⨯=⨯⨯=λm or oA 12.1=λ (b)()()()1927106.12.11067.12--⨯⨯=p 2310532.2-⨯=kg-m/s1123341062.210532.210625.6---⨯=⨯⨯=λm or oA 262.0=λ_______________________________________2、8()03885.00259.02323=⎪⎭⎫⎝⎛==kT E avg eVNowavg avg mE p 2=()()()1931106.103885.01011.92--⨯⨯= or2510064.1-⨯=avg p kg-m/sNow9253410225.610064.110625.6---⨯=⨯⨯==p h λm oroA 25.62=λ_______________________________________2、9pp p hch E λν==Nowmp E ee 22= and221⎪⎪⎭⎫ ⎝⎛=⇒=ee e e h m E hp λλ Set e p E E = and e p λλ10=Then22102121⎪⎪⎭⎫ ⎝⎛=⎪⎪⎭⎫ ⎝⎛=p ep h m hm hcλλλ which yieldsmchp 2100=λ100221002mc mc h hc hc E E p p =⋅===λ ()()1001031011.922831⨯⨯=-151064.1-⨯=J 25.10=keV _______________________________________2、10(a) 1034108510625.6--⨯⨯==λhp2610794.7-⨯= kg-m/s431261056.81011.910794.7⨯=⨯⨯==--m p υm/s or 61056.8⨯=υcm/s()()243121056.81011.92121⨯⨯==-υm E211033.3-⨯=Jor 219211008.2106.110334.3---⨯=⨯⨯=E eV (b) ()()23311081011.921⨯⨯=-E2310915.2-⨯=Jor 419231082.1106.110915.2---⨯=⨯⨯=E eV ()()3311081011.9⨯⨯==-υm p2710288.7-⨯=kg-m/s827351009.910288.710625.6---⨯-⨯⨯==p h λmor oA 909=λ_______________________________________2、11(a) ()()1083410110310625.6--⨯⨯⨯===λνhch E 151099.1-⨯=J Now1915106.11099.1--⨯⨯==⇒⋅=e E V V e E41024.1⨯=V V 4.12=kV (b)()()15311099.11011.922--⨯⨯==mE p231002.6-⨯=kg-m/s Then1123341010.11002.610625.6---⨯=⨯⨯==p h λm oroA 11.0=λ_______________________________________2、126341010054.1--⨯=∆=∆x p 2810054.1-⨯=kg-m/s_______________________________________2、13(a) (i) =∆∆x p26103410783.8101210054.1---⨯=⨯⨯=∆p kg-m/s (ii)p m p dp d p dp dE E ∆⋅⎪⎪⎭⎫⎝⎛=∆⋅=∆22 mpp p m p ∆=∆⋅=22 Now mE p 2=()()()1931106.1161092--⨯⨯= 2410147.2-⨯=kg-m/s so ()()31262410910783.8101466.2---⨯⨯⨯=∆E1910095.2-⨯=Jor 31.1106.110095.21919=⨯⨯=∆--E eV(b) (i) 2610783.8-⨯=∆p kg-m/s (ii)()()()1928106.1161052--⨯⨯=p 231006.5-⨯=kg-m/s()()28262310510783.81006.5---⨯⨯⨯=∆E 2110888.8-⨯=Jor 219211055.5106.110888.8---⨯=⨯⨯=∆E eV _______________________________________2、143223410054.11010054.1---⨯=⨯=∆=∆x p kg-m/s150010054.132-⨯=∆=∆⇒=m p m p υυ 36107-⨯=∆υm/s_______________________________________2、15(a) =∆∆t E()()1619341023.8106.18.010054.1---⨯=⨯⨯=∆t s (b) 1034105.110054.1--⨯⨯=∆=∆x p 251003.7-⨯=kg-m/s_______________________________________2、16(a) If ()t x ,1ψ and ()t x ,2ψ are solutionstoSchrodinger's wave equation, then()()()()t t x j t x x V x t x m ∂ψ∂=ψ+∂ψ∂⋅-,,,2112122 and()()()()t t x j t x x V x t x m ∂ψ∂=ψ+∂ψ∂⋅-,,,2222222 Adding the two equations, we obtain()()[]t x t x x m ,,221222ψ+ψ∂∂⋅- ()()()[]t x t x x V ,,21ψ+ψ+()()[]t x t x tj ,,21ψ+ψ∂∂=which is Schrodinger's wave equation 、 So ()()t x t x ,,21ψ+ψ is also a solution 、(b) If ()()t x t x ,,21ψ⋅ψ were a solution toSchrodinger's wave equation, then we could write []()[]21212222ψ⋅ψ+ψ⋅ψ∂∂⋅-x V x m[]21ψ⋅ψ∂∂=tjwhich can be written as⎥⎦⎤⎢⎣⎡∂ψ∂⋅∂ψ∂+∂ψ∂ψ+∂ψ∂ψ-x x x x m 2121222221222()[]⎥⎦⎤⎢⎣⎡∂ψ∂ψ+∂ψ∂ψ=ψ⋅ψ+t t j x V 122121 Dividing by 21ψ⋅ψ, we find⎥⎦⎤⎢⎣⎡∂ψ∂∂ψ∂ψψ+∂ψ∂⋅ψ+∂ψ∂⋅ψ-x x x xm21212121222222112 ()⎥⎦⎤⎢⎣⎡∂ψ∂ψ+∂ψ∂ψ=+t t j x V 112211Since 1ψ is a solution, then()tj x V x m ∂ψ∂⋅ψ⋅=+∂ψ∂⋅ψ⋅-1121212112Subtracting these last two equations, we have⎥⎦⎤⎢⎣⎡∂ψ∂∂ψ∂ψψ+∂ψ∂⋅ψ-x x x m 212122222212t j ∂ψ∂⋅ψ⋅=221 Since 2ψ is also a solution, we have()t j x V x m ∂ψ∂⋅ψ⋅=+∂ψ∂⋅ψ⋅-2222222112 Subtracting these last two equations, we obtain()02221212=-∂ψ∂⋅∂ψ∂⋅ψψ⋅-x V xx mThis equation is not necessarily valid, which means that 21ψψ is, in general, not a solutionto Schrodinger's wave equation 、_______________________________________2、1712cos 2312=⎪⎭⎫⎝⎛⎰+-dx x A π()12sin 2312=⎥⎦⎤⎢⎣⎡++-ππx x A 121232=⎥⎦⎤⎢⎣⎡⎪⎭⎫ ⎝⎛--Aso 212=Aor 21=A_______________________________________2、18()1cos 22/12/12=⎰+-dx x n A π()142sin 22/12/12=⎥⎦⎤⎢⎣⎡++-ππn x n x A ⎪⎭⎫⎝⎛==⎥⎦⎤⎢⎣⎡⎪⎭⎫ ⎝⎛--211414122A Aor 2=A_______________________________________2、19Note that 10*=ψ⋅ψ⎰∞dxFunction has been normalized 、 (a) Nowdx a x a P oa o o 24exp 2⎰⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛-=dx a x a oa o o⎰⎪⎪⎭⎫⎝⎛-=42exp 2402exp 22o a o o o a x a a ⎪⎪⎭⎫ ⎝⎛-⎪⎪⎭⎫⎝⎛-=or()⎪⎭⎫ ⎝⎛--=⎥⎥⎦⎤⎢⎢⎣⎡-⎪⎪⎭⎫⎝⎛--=21exp 1142exp 1o oa a P which yields 393.0=P (b)dx a x a P o oa a o o 224exp 2⎰⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛-=dx a x a o oa a o o⎰⎪⎪⎭⎫⎝⎛-=242exp 22exp 22o oa a o o o a x a a ⎪⎪⎭⎫ ⎝⎛-⎪⎪⎭⎫⎝⎛-=or()()⎥⎦⎤⎢⎣⎡⎪⎭⎫ ⎝⎛----=21exp 1exp 1Pwhich yields239.0=P (c)dx a x a P oa o o 20exp 2⎰⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛-= dx a x a oa o o⎰⎪⎪⎭⎫⎝⎛-=2exp 2o a o o oa x a a 02exp 22⎪⎪⎭⎫ ⎝⎛-⎪⎪⎭⎫⎝⎛-=()()[]12exp 1---= which yields 865.0=P_______________________________________2、20()dx x P 2⎰=ψ(a)dx x a a ⎪⎭⎫⎝⎛⎪⎭⎫ ⎝⎛⎰2cos 224/0π 4/042sin 22a a a x x a ⎥⎥⎥⎥⎦⎤⎢⎢⎢⎢⎣⎡⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛+⎪⎭⎫ ⎝⎛=ππ⎥⎥⎥⎥⎦⎤⎢⎢⎢⎢⎣⎡⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛+⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛=a a a ππ42sin 242 ()()⎥⎦⎤⎢⎣⎡+⎪⎭⎫ ⎝⎛=π4182a a a or 409.0=P(b) dx a x a P a a ⎪⎭⎫⎝⎛⎪⎭⎫ ⎝⎛=⎰π2/4/2cos 2 2/4/42sin 22a a a a x x a ⎥⎥⎥⎥⎦⎤⎢⎢⎢⎢⎣⎡⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛+⎪⎭⎫ ⎝⎛=ππ()⎥⎥⎥⎥⎦⎤⎢⎢⎢⎢⎣⎡⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛--⎪⎭⎫ ⎝⎛+⎪⎭⎫ ⎝⎛=a a a a a ππππ42sin 84sin 42 ⎥⎦⎤⎢⎣⎡--+=π41810412or 0908.0=P(c) dx a x a P a a ⎪⎭⎫⎝⎛⎪⎭⎫ ⎝⎛=⎰+-π22/2/cos 2 2/2/42sin 22a a a a x x a +-⎥⎥⎥⎥⎦⎤⎢⎢⎢⎢⎣⎡⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛+⎪⎭⎫ ⎝⎛=ππ()()⎥⎥⎥⎥⎦⎤⎢⎢⎢⎢⎣⎡⎪⎭⎫ ⎝⎛--⎪⎭⎫ ⎝⎛--⎪⎭⎫ ⎝⎛+⎪⎭⎫ ⎝⎛=a a a a a ππππ4sin 44sin 42 or 1=P_______________________________________2、21(a) dx a x a P a ⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛=⎰π2sin 224/04/0244sin 22a a a x x a ⎥⎥⎥⎥⎦⎤⎢⎢⎢⎢⎣⎡⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛-⎪⎭⎫ ⎝⎛=ππ()⎥⎥⎥⎥⎦⎤⎢⎢⎢⎢⎣⎡⎪⎭⎫ ⎝⎛-⎪⎭⎫ ⎝⎛=a a a ππ8sin 82or 25.0=P(b) dx a x a P a a ⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛=⎰π2sin 222/4/ 2/4/244sin 22a a a a x x a ⎥⎥⎥⎥⎦⎤⎢⎢⎢⎢⎣⎡⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛-⎪⎭⎫ ⎝⎛=ππ()()⎥⎥⎥⎥⎦⎤⎢⎢⎢⎢⎣⎡⎪⎭⎫ ⎝⎛+⎪⎭⎫ ⎝⎛-⎪⎭⎫ ⎝⎛-⎪⎭⎫ ⎝⎛=a a a a a ππππ8sin 882sin 42 or 25.0=P(c) dx a x a P a a ⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛=⎰+-π2sin 222/2/ 2/2/244sin 22a a a a x x a +-⎥⎥⎥⎥⎦⎤⎢⎢⎢⎢⎣⎡⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛-⎪⎭⎫ ⎝⎛=ππ()()⎥⎥⎥⎥⎦⎤⎢⎢⎢⎢⎣⎡⎪⎭⎫ ⎝⎛-+⎪⎭⎫ ⎝⎛--⎪⎭⎫ ⎝⎛-⎪⎭⎫ ⎝⎛=a a a a a ππππ82sin 482sin 42 or 1=P_______________________________________2、22(a) (i) 481210108108=⨯⨯==k p ωυm/s or 610=p υcm/s9810854.710822-⨯=⨯==ππλk mor oA 54.78=λ (ii)()()431101011.9-⨯==υm p271011.9-⨯=kg-m/s()()24312101011.92121-⨯==υm E2310555.4-⨯=Jor 419231085.2106.110555.4---⨯=⨯⨯=E eV (b) (i) 491310105.1105.1-=⨯-⨯==k p ωυm/s or 610-=p υcm/s991019.4105.122-⨯=⨯==ππλk m or oA 9.41=λ(ii) 271011.9-⨯-=p kg-m/s41085.2-⨯=E eV_______________________________________2、23(a) ()()t kx j Ae t x ω+-=ψ,(b) ()()21921106.1025.0υm E =⨯=- ()2311011.921υ-⨯= so 41037.9⨯=υm/s 61037.9⨯=cm/sFor electron traveling in x -direction, 61037.9⨯-=υcm/s()()4311037.91011.9⨯-⨯==-υm p2610537.8-⨯-=kg-m/s926341076.710537.810625.6---⨯=⨯⨯==p h λm8910097.81076.722⨯=⨯==-πλπk m 1- ()()481037.910097.8⨯⨯=⋅=υωkor 1310586.7⨯=ωrad/s_______________________________________2、24(a) ()()4311051011.9⨯⨯==-υm p 2610555.4-⨯=kg-m/s8263410454.110555.410625.6---⨯=⨯⨯==p h λm881032.410454.122⨯=⨯==-πλπk m 1- ()()481051032.4⨯⨯==υωk131016.2⨯=rad/s (b) ()()631101011.9-⨯=p251011.9-⨯=kg-m/s1025341027.71011.910625.6---⨯=⨯⨯=λm 9101064.810272.72⨯=⨯=-πk m 1- ()()15691064.8101064.8⨯=⨯=ωrad/s _______________________________________2、25()()()2103122342222210751011.9210054.12---⨯⨯⨯==ππn ma n E n()212100698.1-⨯=n E n Jor()19212106.1100698.1--⨯⨯=n E nor ()3210686.6-⨯=n E n eV Then311069.6-⨯=E eV221067.2-⨯=E eV231002.6-⨯=E eV_______________________________________2、26(a) ()()()2103122342222210101011.9210054.12---⨯⨯⨯==ππn ma n E n ()20210018.6-⨯=n J or()()3761.0106.110018.6219202n n E n =⨯⨯=--eV Then376.01=E eV 504.12=E eV 385.33=E eV(b) Ehc ∆=λ ()()19106.1504.1385.3-⨯-=∆E191001.3-⨯=J()()198341001.310310625.6--⨯⨯⨯=λ710604.6-⨯=m or 4.660=λnm_______________________________________2、27(a) 22222ma n E n π =()()()223223423102.11015210054.11015----⨯⨯⨯=⨯πn()622310538.21015--⨯=⨯n or 2910688.7⨯=n (b) 151≅+n E mJ (c) No_______________________________________2、28For a neutron and 1=n :()()()2142722342221101066.1210054.12---⨯⨯==ππma E13103025.3-⨯=Jor6191311006.2106.1103025.3⨯=⨯⨯=--E eV For an electron in the same potential well: ()()()2143122341101011.9210054.1---⨯⨯=πE10100177.6-⨯=J or9191011076.3106.1100177.6⨯=⨯⨯=--E eV _______________________________________2、29Schrodinger's time-independent waveequation()()()()02222=-+∂∂x x V E mx x ψψWe know that()0=x ψ for 2a x ≥ and 2ax -≤We have()0=x V for 22a x a +<<-so in this region()()02222=+∂∂x mEx x ψψThe solution is of the form ()kx B kx A x sin cos +=ψ where22 mEk =Boundary conditions:()0=x ψ at 2,2a x a x +=-= First mode solution: ()x k A x 111cos =ψ where222112ma E a k ππ=⇒=Second mode solution: ()x k B x 222sin =ψ where22222242ma E a k ππ=⇒= Third mode solution: ()x k A x 333cos =ψ where22233293ma E a k ππ=⇒= Fourth mode solution: ()x k B x 444sin =ψ where222442164ma E a k ππ=⇒= _______________________________________2、30The 3-D time-independent wave equation incartesian coordinates for ()0,,=z y x V is:()()()222222,,,,,,z z y x y z y x x z y x ∂∂+∂∂+∂∂ψψψ ()0,,22=+z y x mEψUse separation of variables, so let ()()()()z Z y Y x X z y x =,,ψSubstituting into the wave equation, we obtain222222zZXY y Y XZ x X YZ ∂∂+∂∂+∂∂ 022=+XYZ mEDividing by XYZ and letting222 mEk =, wefind(1) 01112222222=+∂∂⋅+∂∂⋅+∂∂⋅k zZ Z y Y Y x X XWe may set01222222=+∂∂⇒-=∂∂⋅X k xX k x X X x x Solution is of the form()()()x k B x k A x X x x cos sin += Boundary conditions: ()000=⇒=B X and ()an k a x X x x π=⇒==0 where ....3,2,1=x n Similarly, let2221y k y Y Y -=∂∂⋅ and 2221z k zZ Z -=∂∂⋅Applying the boundary conditions, we finda n k y y π=, ....3,2,1=y nan k z z π=, ...3,2,1=z nFrom Equation (1) above, we have02222=+---k k k k z y x or222222 mEk k k k z y x ==++so that()2222222z y x n n n n n n maE E z y x ++=→π _______________________________________2、31 (a)()()()0,2,,22222=⋅+∂∂+∂∂y x mEy y x x y x ψψψSolution is of the form:()y k x k A y x y x sin sin ,⋅=ψWe find()y k x k Ak x y x y x x sin cos ,⋅=∂∂ψ ()y k x k Ak xy x y x x sin sin ,222⋅-=∂∂ψ()y k x k Ak y y x y x y cos sin ,⋅=∂∂ψ()y k x k Ak yy x y x y sin sin ,222⋅-=∂∂ψSubstituting into the original equation, we find:(1) 02222=+--mE k k y xFrom the boundary conditions, 0sin =a k A x , where oA a 40= So an k x x π=, ...,3,2,1=x n Also 0sin =b k A y , where oA b 20= So bn k y y π=, ...,3,2,1=y n Substituting into Eq 、 (1) above⎪⎪⎭⎫ ⎝⎛+=22222222b n an m E y x n n yx ππ (b)Energy is quantized - similar to 1-D result 、There can be more than one quantum stateper given energy - different than 1-D result 、_______________________________________2、32(a) Derivation of energy levels exactly thesame as in the text(b) ()21222222n n maE -=∆π For 1,212==n n Then22223ma E π =∆(i) For oA a 4= ()()()2102722341041067.1210054.13---⨯⨯⨯=∆πE2210155.6-⨯=Jor 319221085.3106.110155.6---⨯=⨯⨯=∆E eV(ii) For 5.0=a cm()()()22272234105.01067.1210054.13---⨯⨯⨯=∆πE3610939.3-⨯=Jor1719361046.2106.110939.3---⨯=⨯⨯=∆E eV _______________________________________2、33(a) For region II, 0>x()()()0222222=-+∂∂x V E mx x O ψψGeneral form of the solution is()()()x jk B x jk A x 22222exp exp -+=ψ where()O V E mk -=222 Term with 2B represents incident wave andterm with 2A represents reflected wave 、 Region I, 0<x()()0212212=+∂∂x mEx x ψψGeneral form of the solution is()()()x jk B x jk A x 11111exp exp -+=ψ where212 mEk =Term involving 1B represents the transmitted wave and the term involving 1A represents reflected wave: but if a particle is transmitted into region I, it will not be reflected so that 01=A 、 Then()()x jk B x 111exp -=ψ()()()x jk B x jk A x 22222exp exp -+=ψ (b)Boundary conditions: (1) ()()0021===x x ψψ(2) 0201==∂∂=∂∂x x x x ψψ Applying the boundary conditions to the solutions, we find221B A B +=112222B k B k A k -=-Combining these two equations, we find212122B k k k k A ⋅⎪⎪⎭⎫⎝⎛+-=212212B k k k B ⋅⎪⎪⎭⎫⎝⎛+=The reflection coefficient is21212*22*22⎪⎪⎭⎫ ⎝⎛+-==k k k k B B A A R The transmission coefficient is()2212141k k k k T R T +=⇒-=_______________________________________2、34()()x k A x 222exp -=ψ()()x k A A x P 2*2222exp -==ψwhere ()222 E V m k o -=()()()34193110054.1106.18.25.31011.92---⨯⨯-⨯=9210286.4⨯=k m 1- (a) For 101055-⨯==oA x m ()x k P 22exp -=()()[]109105102859.42exp -⨯⨯-= 0138.0=(b) For 10101515-⨯==oA x m()()[]1091015102859.42exp -⨯⨯-=P61061.2-⨯= (c) For 10104040-⨯==oA x m()()[]1091040102859.42exp -⨯⨯-=P151029.1-⨯=_______________________________________2、35()a k VE VET o o22exp 116-⎪⎪⎭⎫⎝⎛-⎪⎪⎭⎫ ⎝⎛≅ where ()222 E V m k o -=()()()34193110054.1106.11.00.11011.92---⨯⨯-⨯=or 2k 910860.4⨯=m 1-(a) For 10104-⨯=a m()()[]1091041085976.42exp 0.11.010.11.016-⨯⨯-⎪⎭⎫ ⎝⎛-⎪⎭⎫ ⎝⎛≅T 0295.0=(b) For 101012-⨯=a m()()[]10910121085976.42exp 0.11.010.11.016-⨯⨯-⎪⎭⎫ ⎝⎛-⎪⎭⎫ ⎝⎛≅T 51024.1-⨯=(c) υe N J t =, where t N is the density of transmitted electrons 、 1.0=E eV 20106.1-⨯=J ()23121011.92121υυ-⨯==m510874.1⨯=⇒υm/s 710874.1⨯=cm/s()()719310874.1106.1102.1⨯⨯=⨯--t N810002.4⨯=t N electrons/cm 3 Density of incident electrons,10810357.10295.010002.4⨯=⨯=i N cm 3-_______________________________________2、36()a k VEV E T O O 22exp 116-⎪⎪⎭⎫⎝⎛-⎪⎪⎭⎫ ⎝⎛≅ (a) For ()o m m 067.0=()222 E V m k O -=()()()()()2/1234193110054.1106.12.08.01011.9067.02⎪⎭⎪⎬⎫⎪⎩⎪⎨⎧⨯⨯-⨯=---or9210027.1⨯=k m 1- Then⎪⎭⎫⎝⎛-⎪⎭⎫ ⎝⎛=8.02.018.02.016T()()[]109101510027.12exp -⨯⨯-⨯ or138.0=T (b) For ()o m m 08.1=2k =()()()()()2/1234193110054.1106.12.08.01011.908.12⎪⎭⎪⎬⎫⎪⎩⎪⎨⎧⨯⨯-⨯---or9210124.4⨯=k m 1- Then⎪⎭⎫⎝⎛-⎪⎭⎫ ⎝⎛=8.02.018.02.016T()()[]109101510124.42exp -⨯⨯-⨯or51027.1-⨯=T_______________________________________2、37()a k VE VET o o22exp 116-⎪⎪⎭⎫⎝⎛-⎪⎪⎭⎫ ⎝⎛≅ where ()222 E V m k o -=()()()341962710054.1106.1101121067.12---⨯⨯⨯⨯-⨯=1410274.7⨯=m 1- (a)()()[]14141010274.72exp 121112116-⨯-⎪⎭⎫ ⎝⎛-⎪⎭⎫ ⎝⎛≅T[]548.14exp 222.1-=710875.5-⨯= (b)()()710875.510-⨯=T()[]a 1410274.72exp 222.1⨯-=()⎪⎭⎫⎝⎛⨯=⨯-61410875.5222.1ln 10274.72a or 1410842.0-⨯=a m_______________________________________2、38Region I ()0<x , 0=V ;Region II ()a x <<0, O V V = Region III ()a x >, 0=V (a) Region I:()()()x jk B x jk A x 11111exp exp -+=ψ(incident) (reflected)where212 mEk =Region II:()()()x k B x k A x 22222exp exp -+=ψwhere ()222 E V m k O -=Region III:()()()x jk B x jk A x 13133exp exp -+=ψ (b)In Region III, the 3B term represents areflected wave 、 However, once a particleis transmitted into Region III, there will not be a reflected wave so that 03=B 、(c) Boundary conditions:At 0=x : ⇒=21ψψ2211B A B A +=+ ⇒=dx d dx d 21ψψ22221111B k A k B jk A jk -=-At a x =: ⇒=32ψψ()()a k B a k A 2222exp exp -+()a jk A 13exp =⇒=dxd dx d 32ψψ()()a k B k a k A k 222222exp exp -- ()a jk A jk 131exp = The transmission coefficient is defined as*11*33A A A A T = so from the boundary conditions, wewant to solve for 3A in terms of 1A 、Solvingfor 1A in terms of 3A , we find(){()()[]a k a k k k k k jA A 2221222131exp exp 4---+= ()()[]}a k a k k jk 2221exp exp 2-+-()a jk 1exp ⨯We then find()(){()[a k k k k k A A A A 22122221*33*11exp 4-=()]22exp a k --()()[]}2222221exp exp 4a k a k k k -++ We have()222 E V m k O -= If we assume that E V O >>, then a k 2 will be large so that ()()a k a k 22exp exp ->> We can then write()(){()[]222122221*33*11exp 4a k k k k k A A A A -= ()[]}222221exp 4a k k k + which becomes()()()a k k k k k A A A A 22122221*33*112exp 4+= Substituting the expressions for 1k and2k , we find222212 O mV k k =+ and()⎥⎦⎤⎢⎣⎡⎥⎦⎤⎢⎣⎡-=22222122 mE E V m k k O ()()E E V m O -⎪⎭⎫ ⎝⎛=222()()E V E V m O O ⎪⎪⎭⎫ ⎝⎛-⎪⎭⎫⎝⎛=1222 Then()()⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛-⎪⎭⎫ ⎝⎛⎪⎪⎭⎫⎝⎛=E V E V m a k mV A A A A O O O12162exp 222222*33*11()a k V EV E A A O O 2*332exp 116-⎪⎪⎭⎫ ⎝⎛-⎪⎪⎭⎫ ⎝⎛=Finally,()a k V E V E A A A A T O O 2*11*332exp 116-⎪⎪⎭⎫ ⎝⎛-⎪⎪⎭⎫ ⎝⎛== _____________________________________2、39 Region I: 0=V ()()⇒=+∂∂0212212x mE x x ψψ()()()x jk B x jk A x 11111exp exp -+=ψincidentreflectedwhere212 mEk = Region II: 1V V = ()()()⇒=-+∂∂02221222x V E m x x ψψ ()()()x jk B x jk A x 22222exp exp -+=ψ transmitted reflectedwhere()2122V E m k -= Region III: 2V V =()()()⇒=-+∂∂02322232x V E m x x ψψ()()x jk A x 333exp =ψtransmitted where ()2232 V E m k -=There is no reflected wave in Region III 、 The transmission coefficient is defined as:*11*3313*11*3313A A A A k k A A A A T ⋅=⋅=υυ From the boundary conditions, solve for 3Ain terms of 1A 、 The boundary conditions are:At 0=x : ⇒=21ψψ2211B A B A +=+⇒∂∂=∂∂xx 21ψψ22221111B k A k B k A k -=-At a x =: ⇒=32ψψ ()()a jk B a jk A 2222exp exp -+ ()a jk A 33exp =⇒∂∂=∂∂xx 32ψψ()()a jk B k a jk A k 222222exp exp --()a jk A k 333exp = But ⇒=πn a k 22 ()()1exp exp 22=-=a jk a jk Then, eliminating 1B , 2A , 2B from the boundary condition equations, we find()()23131231211344k k k k k k k k k T +=+⋅= _______________________________________2、40 (a) Region I: Since E V O >, we can write()()()0212212=--∂∂x E V m x x O ψψRegion II: 0=V , so()()0222222=+∂∂x mEx x ψψRegion III: 03=⇒∞→ψV The general solutions can be written, keeping in mind that 1ψ must remain finite for 0<x , as()()x k B x 111exp =ψ()()()x k B x k A x 22222cos sin +=ψ ()03=x ψ where()212 E V m k O -= and222 mEk =(b) Boundary conditionsAt 0=x : ⇒=21ψψ21B B = 221121A k B k xx =⇒∂∂=∂∂ψψ At a x =: ⇒=32ψψ ()()0cos sin 2222=+a k B a k A or()a k A B 222tan -= (c)12122211B k k A A k B k ⎪⎪⎭⎫⎝⎛=⇒=and since 21B B =, then2212B k k A ⎪⎪⎭⎫⎝⎛=From ()a k A B 222tan -=, we can write ()a k B k k B 22212tan ⎪⎪⎭⎫⎝⎛-=or()a k k k 221tan 1⎪⎪⎭⎫⎝⎛-=This equation can be written as⎥⎥⎦⎤⎢⎢⎣⎡⋅⋅--=a mE E EV O 22tan 1 or⎥⎥⎦⎤⎢⎢⎣⎡⋅-=-a mE E V EO 22tan This last equation is valid only forspecific values of the total energy E 、 The energy levels are quantized 、_______________________________________2、41 ()222424ne m E o o n ∈-=π(J)()222324n e m o o ∈-=π(eV)()()()[]()22342123193110054.121085.84106.11011.9n----⨯⨯⨯⨯-=πor258.13n E n -= (eV) 58.1311-=⇒=E n eV 395.322-=⇒=E n eV 51.133-=⇒=E n eV 849.044-=⇒=E n eV_______________________________________2、42We have⎪⎪⎭⎫⎝⎛-⎪⎪⎭⎫⎝⎛⋅=o oa r a exp 112/3100πψ and*10010024ψψπr P =⎪⎪⎭⎫ ⎝⎛-⎪⎪⎭⎫⎝⎛⋅⋅=o o a r a r 2exp 11432ππ or()⎪⎪⎭⎫ ⎝⎛-⋅=o o a r r a P 2exp 423 To find the maximum probability()0=drr dP()()⎪⎪⎭⎫ ⎝⎛-⎪⎩⎪⎨⎧⎪⎪⎭⎫ ⎝⎛-=o o o a r r a a 2exp 2423 ⎪⎭⎪⎬⎫⎪⎪⎭⎫ ⎝⎛-+o a r r 2exp 2 which giveso oa r a r=⇒+-=10 or o a r = is the radius that gives the greatest probability 、 _______________________________________2、43100ψ is independent of θ and φ, so the wave equation in spherical coordinates reduces to ()()021222=-+⎪⎭⎫ ⎝⎛∂∂∂∂⋅ψψr V E m r r r r o where()r a m r e r V o o o 224 -=∈-=π For ⎪⎪⎭⎫ ⎝⎛-⎪⎪⎭⎫ ⎝⎛⋅=o o a r a exp 112/3100πψ Then ⎪⎪⎭⎫ ⎝⎛-⎪⎪⎭⎫ ⎝⎛-⎪⎪⎭⎫ ⎝⎛⋅=∂∂o o o a r a a r exp 1112/3100πψ so⎪⎪⎭⎫ ⎝⎛-⎪⎪⎭⎫ ⎝⎛⋅-=∂∂o o a r r a r r exp 1122/51002πψ We then obtain2/5100211⎪⎪⎭⎫⎝⎛⋅-=⎪⎪⎭⎫ ⎝⎛∂∂∂∂o a r r r πψ ⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛-⎪⎪⎭⎫ ⎝⎛-⎪⎪⎭⎫ ⎝⎛-⨯o o o a r a r a r r exp exp 22 Substituting into the wave equation, we have⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛--⎪⎪⎭⎫ ⎝⎛-⎪⎪⎭⎫ ⎝⎛⋅-o o o o a r a r a r r a r exp exp 21122/52π⎥⎦⎤⎢⎣⎡++r a m E m o o o 2220exp 112/3=⎪⎪⎭⎫ ⎝⎛-⎪⎪⎭⎫ ⎝⎛⋅⎪⎪⎭⎫ ⎝⎛⨯o o a r a π where ()222241224oo o o a m e m E E -=∈-==π Then the above equation becomes⎪⎩⎪⎨⎧⎥⎦⎤⎢⎣⎡--⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛-⎪⎪⎭⎫ ⎝⎛⋅o o o o a r r a r a r a 222/321exp 11π 022222=⎪⎭⎪⎬⎫⎪⎪⎭⎫ ⎝⎛+-+r a m a m m o o o o oor ⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛-⎪⎪⎭⎫ ⎝⎛⋅o o a r a exp 112/3π0211222=⎪⎭⎪⎬⎫⎪⎩⎪⎨⎧⎪⎪⎭⎫ ⎝⎛+-++-⨯r a a a r a o o o o which gives 0 = 0 and shows that 100ψ is indeed a solution to the wave equation 、 _______________________________________ 2、44All elements are from the Group I column ofthe periodic table 、 All have one valence electron in the outer shell 、 _______________________________________。

半导体物理与器件第四版课后习题答案(供参考).doc

Chapter 44.1n i 2E gN c N expkTT 3E gexpN cO N O300kTwhere N cO and N Oare the values at 300 K.(a) SiliconT (K) kT (eV) n i (cm 3) 200 0.01727 7.68 104 400 0.03453 2.38 1210 6000.05189.74 1014(c) GaAs(b) GermaniumT (K)n i (cm 3 ) n i (cm 3 ) 200 2.16 10101.38 4008.60 1014 3.28 109 6003.82 10165.72 1012_______________________________________ 4.2Plot_______________________________________4.3(a) n i 2 N c NexpE gkT31121919T5 2.8 1.04 101010300exp1.120.0259 T 300T 32.5 10 232.912 10 38300exp1.12 3000.0259 TBy trial and error, T 367.5 K(b)n i25 10 1222.5 10 2532.912 10 38T exp 1.12 300300 0.0259 TBy trial and error,T 417.5 K _______________________________________4.4At T200 K, kT0.02592003000. 017267eVAt T400 K, kT0.02594003000. 034533eVn i 2400 7.70 101023.025 10 17n i 2 2001.40 10 2 23400expE g3000.0345333200Egexp300 0.017267E gE g8 exp0.0345330.0172673.025 10178 exp E g 57 .9139 28.9578orE g 28.9561ln 3.025 1017 38.17148 or E g 1.318 eVNow7.70 1010N co N o340023001.318 exp0.03453321N co N o 2.370 175.929 10 2.658 10so N co N o 9.41 10 37 cm 6_______________________________________4.5exp 1.10n i kT 0.20Bexpn i A 0.90 kTexp kTFor T 200 K, kT 0.017267 eVFor T 300 K, kT 0.0259 eVFor T 400 K, kT 0.034533 eV(a) For T 200K,n i B exp 0.20 9.325 10 6n i A 0.017267(b) For T 300K,n i Bexp 0.204.43 10 4n i A 0.0259 (c) For T 400K,n i Bexp 0.203.05 10 3n i A 0.034533_______________________________________ 4.6(a) g c f FE E FE E c expkTThen g c f F x expxkTTo find the maximum value:d g c f F 1 x1 / 2 exp xdx 2 kT1 x1 /2 exp x 0kT kTwhich yields1/ 21 x kT2x1/ 2 x 2kTThe maximum value occurs atEkTE c2(b)g 1 f FE F EE E expkTE EE E expkTexpE F EkTLet E E xThen g 1 f F x expxkTTo find the maximum valued g 1 f F d xdx dxx expkTSame as part (a). Maximum occurs atxkT2E E c exp E E ckTorkTE E2E c EF expkTLet E E c x _______________________________________ 4.7E1 E c exp E1 E cn E1 kTn E2E2 E c exp E2 E c kTwhereE1 E c 4kT and E 2 E c kT 2Thenn E1 4kTexp E1 E2n E2 kT kT22 2 exp 4 12 exp 3.522orn E10.0854n E 2_______________________________________ 4.8Plot_______________________________________4.9Plot_______________________________________ 4.10E Fi E midgap 3kT ln m*pm n* 4Silicon: m*p 0.56 m o , m n* 1.08m oE Fi E midgap 0.0128 eVGermanium: m*p 0. 37m o ,*0.55m om nE Fi E midgap 0 .0077 eVGallium Arsenide: m*p 0.48m o ,m n* 0.067m oE Fi E midgap 0 .0382 eV_______________________________________ 4.11E Fi E midgap 1 kT ln N2 N c1kT ln 1.04 1019 0.4952 kT2 2.8 1019T (K) kT (eV) ( E Fi E midgap )(eV) 200 0.01727 0.0086 400 0.03453 0.0171 600 0.0518 0.0257_______________________________________4.12(a) E Fi E midgapm*p3 kT ln4 m n*3 0.0259 ln0.704 1.2110.63 meV(b) E Fi E midgap 3 0.0259 ln0.754 0.08043.47 meV_______________________________________4.13Let g c E K constantThenn o g c E f F E dEE cK1dEE E FEc 1 expkTK expE E FdEkTE cLetE E cso that dE kT dkTWe can writeE EF E c E F E E cso thatE E Fexp E c E FexpexpkTkTThe integral can then be written asn o K kT exp E c E Fexp d kTwhich becomesn o K kTE c EF expkT_______________________________________4.14Let g c E C1E E c for E E cThenn o g c E f F E dEE cC1 E E cdEE c 1exp E EF kTC1 EE E FdE E C expE ckTLetE E cdE kT dso thatkTWe can writeE EF E E c E c E FThenE c E Fn o C1 expkTE E cE E cdE expE ckT orn oE c EF C1 expkTkT exp kT d 0We find thatexp d exp 1 1So2 E c E Fn o C1 kT expkT_______________________________________4.15r1 m oWe have rm*a oFor germanium, r 16 , m* 0.55m oThenr1 16 1 a o 29 0.530.55oror1 15.4 AThe ionization energy can be written asm*2E o 13.6 eVm o s0.552 13.6 E 0.029 eV16_______________________________________ 4.16We have r1 m orm*a oFor gallium arsenide, r 13.1 , *m0.067 m o1or1 13.1 104 A0.530.067The ionization energy ism*20.067E o 13.6 13.6m o s 13.1 2orE0.0053 eV_______________________________________4.17Nc(a) E c E F kT ln2.8 10190.0259 ln 157 100.2148 eV(b) E F E E g E c E F1.12 0.2148 0.90518eV(c) p o NE F E expkT1.04 19 0.9051810 exp0.02596.90 103cm 3(d) Holesn o(e) E F E Fi kT lnn i710 150.0259 ln1.5 10100.338 eV_______________________________________4.18N(a) E F E kT lnp o190.0259 ln 1.0410210160.162 eV(b) E c E F E g E F E1.12 0.162 0.958 eV(c) n o 2.8 19 0.95810 exp0.02592.41 103cm3p o(d) E Fi E F kT lnn i2 10 160.0259 ln 101.5 100.365eV_______________________________________4.19Nc(a) E c E F kT ln0.0259 ln 2.810192 1050.8436 eVE F E E g E c E F1.12 0.8436E F E 0.2764 eV(b) p o 1.04 1019 exp 0.276370.02592.414 1014cm3(c)p-type_______________________________________4.20(a) kT3750.032375 eV0.02593003 / 2n o 4.7 10 17 375 exp 0.28300 0.0323751.15 1014cm3E F E E g E c E F 1.42 0.281.14 eV375 3 / 2 1.14 p o 7 18 exp10300 0.0323754.99 103cm 3(b) E c E F 0.0259 ln 4.7 10171.15 10 140.2154 eVE F E E g E c E F 1.42 0.21541.2046 eVp o 7 10 18 exp 1.20460.02594.42 10 2cm 3_______________________________________ 4.21(a) kT 0.0259 3750.032375 eV 300375 3 / 2 0.28n o 2.8 19 exp10300 0.0323756.86 1015cm 3E F E E g E c E F 1.12 0.280.840 eV375 3 / 20.840p o 1.04 1019 exp300 0.0323757.84 107cm 3(b) E c E F kT ln N cn o0.0259 ln2.8 10196.862 10 150.2153 eVE F E 1.12 0.2153 0.9047 eVp o 1.04 10 19 exp 0.9046680.02597.04 103 cm 3_______________________________________4.22(a) p-typeE g(b) E F E1.124 0.28 eV4p o N exp E F EkT1.04 10 19 exp 0.280.02592.10 1014cm 3E c EF E g E F E1.12 0.28 0.84 eVn o N c exp E c E FkT2.8 1019exp0.840.02592.30 105cm 3_______________________________________4.23(a) n o n iE F E FiexpkT1.5 1010 exp 0.220.02597.3313cm310p oE Fi E Fn i expkT1.5 1010 exp 0.220.02593.07 106cm 3(b) n o n iE F E FiexpkT1.8 10 6 exp 0.220.02598.80 109cm 3p o n i expE Fi E FkT1.8 106 exp 0.220.02593.68 102cm 3_______________________________________4.24(a) E F ENkT lnp o0.0259 ln1.04 10 195 10 150.1979 eV(b) E c E F E g E F E1.12 0.19788 0.92212 eV(c) n o 2.8 1019 exp 0.922120.02599.66 103cm 3(d) Holesp o(e) E Fi E F kT lnn i510 150.0259 ln1.5 10100.3294 eV _______________________________________4.25kT 0.0259 4000.034533 eV 3003 / 2N 1.04 10 19400300 1.601 1019cm 33 / 2N c 2.8 1019400300 4.3109 1019cm 30.2642 eV _______________________________________4.26(a) p o 7 1018 exp 0.250.02594.50 1014cm 3E c EF 1.42 0.25 1.17 eVn o 4.7 10 17 exp 1.170.02591.13 10 2cm 3(b)kT 0.034533eV3 / 2N 7 10184003001.078 1019cm 33 / 217 400N c 4.7 103007.236 1017cm3expn i 2 4.3109 10 19 1.601 10191.12NE F E kT lnp o19 0.0345335.67022410n i 2.381 1012 cm 3(a) E F ENkT lnp o0.034533 ln 1.601 10195 1015 0.2787 eV(b) E c E F 1.12 0.27873 0.84127 eV(c) n o 4.3109 10 19 exp 0.841270.0345331.134 109cm3(d) Holes(e) E Fi E F kT ln p on i510150.034533 ln2.381 10120.034533 ln1.078104.50 10 140.3482 eVE c EF 1.42 0.3482 1.072 eVn o 7.236 1017 exp 1 .071770. 0345332.40 104cm 3_____________________________________4.27(a) p o 1.04 1019 exp 0.250.02596.68 1014cm 3E c EF 1.12 0.25 0.870 eVn o 2.8 10 19 exp 0.8700.0259n o7.2310 4 cm 3(b)kT0.034533 eV3 / 2N 1.04 10194003001.601 1019cm 33 / 2N c 2.8 1019 4003004.311 1019cm 3NE F E kT lnp o1.60110 190.034533ln6.6810140.3482 eVE c EF 1.12 0.34820.7718 eVn o 4.311 1019 exp 0.771750.0345338.49 109cm 3_______________________________________4.282(a) n o N c F1 / 2 FFor E F E c kT 2 ,E F E c kT 2 FkT 0.5kTThen F1/ 2 F 1.0n o 2 2.8 1019 1.03.16 1019cm 3(b) n o 2 N c F1 / 2 F24.7 1017 1.05.30 1017cm 3_______________________________________ 4.29p o 2 N F1/2 F5 1019 2 1.04 1019 F1/2 FSo F1/ 2 F 4.26We find F 3.0E E FkTE EF 3.0 0.0259 0.0777 eV_______________________________________4.30E F E c 4kT(a) F 4kT kTThen F1 / 2 F 6.02N c F1 / 2n o F2 2.8 1019 6.01.90 10 20 cm 3(b) n o 2 4.7 1017 6.03.18 1018cm 3_______________________________________ 4.31For the electron concentrationn E g c E f F EThe Boltzmann approximation applies, so4 * 3 / 22m nE E cn Eh3E E FexpkTor4 2m n* 3 / 2 E c E Fexpn E h3kTE E c E E ckT expkTkTDefinexEE ckTThenn E n x K x exp xTo find maximumn E n x , setdn x 0 K 1 x 1 / 2 exp xdx 2x 1 / 21 expxorKx 1 / 2 expx1 x2which yieldsx1 E E cE E c12kTkT2For the hole concentrationp Eg E 1f F EUsing the Boltzmann approximation4 2m p * 3 / 2p EEEh 3E F EexpkT or3 / 242m *p E F Ep Eh 3expkTE E E EkTexpkTkTDefinexE EkTThenp xK x exp xTo find maximum value ofp Ep x ,setdp xUsing the results from0 dxabove,we find the maximum at1E E kT2_______________________________________4.32 (a) Silicon:We haven oN c expE cE FkTWe can writeE c E FE c E d E d E FForE c E d 0.045 eV andE dE F3kT eVwe can writen o2.8 1019 exp 0.04530.02592.8 1019exp 4.737or10 17 cm3n o2.45 We also havep oN expE F EkTAgain, we can writeE FEE FE aE aEForE FE a3kTandE aE0.045eVThenp o1.04 1019 exp 3 0.0450.02591.04 1019 exp4.737orp o9.12 10 16 cm 3(b) GaAs: assume E c E d0.0058eVThenn o4.7 1017 exp0.0058 30.025917exp 3.2244.7 10orn o1.87 1016 cm3Assume E a E 0.0345 eVThenp o71018 exp0.0345 30.02597 1018 exp 4.332orp o9.20 1016 cm 3_______________________________________ 4.33Plot_______________________________________4.34 10 151015 cm 3(a)p o415 31.5 10 10 2n o7.5 10 4 cm33 10153(b) n oN d316cm1010 2p o1.5 107.5 10 3cm 33 1016 (c)n op on i 1.5 10 10cm33(d) n i 22.8 10 19 1.041019 375300 exp1.12 3000.0259 375n i7.334 1011 cm3p o N a4 10 15 cm 37.334 10 11 2n o1.34 10 8 cm34 10 153(e) n i 22.8 10 19 1.04 10 19 4503001.12 300exp0.0259 450133n i1.722 10 cm14142n o1.722 10 1310102221.029 1014 cm 31.722 1013 2p o2.88 1012 cm 31.029 1014_______________________________________(a) p oN aN d4 101510153 1015 cm 3n i 2 1.8 10 6 2n o1.08 10 3cm 3p o3 1015(b) n oN d 3 10 16 cm 3p o1.8 10 6 2 1.08 10 4 cm33 10163(c) n o p on i1.8 10 6cm375 3(d) n i 24.7 1017 7.0 10 18300 exp1.42 3000.0259 375n i 7.580 10 8 cm 3p o N a4 1015 cm 38 2n o7.580 10 1.44 10 2 cm 34 10 153 (e) 2 4.7 10 17 7.0 18450 n i 10 300 exp1.42 3000.0259 450n i 3.853 1010 cm3n oN d10 14 cm 33.853 1010 2p o1.48 10 7 cm 310 14_______________________________________4.3610 13 cm 3(a) Ge: n i2.42(i) n oN dN dn i 22 22 10152 210152.4 13 22210or2 1015 cm 3n oN d4.35n i 2 2.4 1013 2p o2 1015n o2.88 1011 cm 3(ii) p o N a N d 10167 10153 1015 cm 32n i22.4 10 13n op o310 151.92 1011cm3(b) GaAs: n i 1.8 10 6cm3(i) n o N d2 1015 cm62p o1.8 10 1.62 10 3cm32 10 15(ii) p oN aN d3 10 15 cm 362n o1.8 101.08 10 3cm 33 1015 (c) The result implies that there is only one 33minority carrier in a volume of 10 cm ._______________________________________4.37(a) For the donor leveln d 1N d1 1exp EdE F2kT11 1 exp 0.2020.0259orn d8.85 10 4N d (b) We havef F E1E E F1expkTNowE E FE E cE c E ForE EF kT 0.245Thenf F E10.2451 exp 1 0.0259orf F E 2.87 10 5_______________________________________4.38N aN d(a) p-type(b) Silicon:10131013p oN aN d 2.5 1 or1013 cm 3p o1.5Thenn i 21.5 10 10 210 7cm 3n o1.5p o 1.5 1013 Germanium:N aN d N a N d 2p o2n i 221.5131.5 10 1322.4 101310222or3.26 10 13 cm 3p oThen2n i 22.4 10 13n o1.76 10 13p o3.264 1013cm 3Gallium Arsenide:p oN a N d1.5 10 13 cm 3and2n i 21.8 10 6n o0.216 cm 3p o1.5 1013_______________________________________4.39 (a) N d N an-type(b) n oN d N a 2 10151.2 10158 1014 cm 3n i 21.5 101022.81 10 5cm 3p o8 14n o10(c)p o N aN a N d4 1015N a 1.2 10 152 1015N a 4.8 10 15 cm31.5 10 102n o5.625 10 4cm 3 4 1015_______________________________________4.40n i21.5 101021. 153n o2 10 5 125 10cmp on o p on-type_______________________________________4.413n i 21.04 10196.0 10 18 250300 exp0.660.0259250 3001.8936 102412n i 1.376cm310 n on i 2 n i 2n o 21n i 2p o4n o 4n o1n i2Son o 6.88 1011 cm 3 ,Then p o2.75 1012cm3N a N a 2p on i 222N a22.752 10122N a21.8936 10 24227.5735 10 242.752 10 12 N aN a2N a 21.8936 10 242so that N a 2.064 1012cm 3_______________________________________4.42Plot_______________________________________4.43Plot_______________________________________4.44Plot_______________________________________ 4.45N d N aN dN a 2n o2n i 2214141.1 1014 2 10 1.2 102 2 10141.2 1014 2n i 221.1 10144 10 1324 10132n i 24.9 10 271.6 10 27n i2so n i5.74 10 13 cm 3p on i 23.3 10 273 133n o 1.1 10 1410 cm_______________________________________4.46(a)N a N d p-typeMajority carriers are holesp o N a N d16163 101.5 101.5 1016 cm 3Minority carriers are electrons210 10 2n on i 1.5 1.5 10 4 cm 3p o 1.5 1016(b) Boron atoms must be addedp o N a N aN d5 1016N a 3 10161.5 1016So N a3.5 10 16 cm 31.5 10 102n o4.5 10 3cm 35 10 16_______________________________________4.47p on i (a)n-type(b) p on i 2 n on i 2n op o1.5 10 1021016 cm3n o4 1.125 2 10electrons are majoritycarriersp o2 10 4cm3holes are minority carriers(c) n oN d N a1.125 101615N d 7 10so N d1.825 1016 cm3_______________________________________4.48E Fi E FkT lnp on iFor GermaniumT (K)kT (eV)n i (cm 3)200 0.01727 2.16 1010400 0.03453 8.60 1410 6000.05183.82 1016N aN a 2p o n i 2and22N a10 15 cm 3T (K)p o (cm3)E Fi EF (eV)200 1.0 1015 0.1855 4001.49 1015 0.01898 6003.87 10160.000674_______________________________________4.49(a) E c E FkT lnN cN d0.0259 ln 2.8 1019N dFor 1014cm 3 , E cE F 0.3249eV15 cm 3 ,E cE F0.2652eV1016cm 3, E c E F 0.2056eV 101017 cm 3 , E c E F0.1459eV(b) E F E FikT lnN dn i0.0259 lnN d1.51010For 1014cm 3 , E FE Fi 0.2280 eV15cm 3, E F E Fi 0.2877 eV10 1016 cm 3 , E F E Fi 0.3473 eV 1017 cm 3 ,E F E Fi0.4070 eV_______________________________________ 4.50N d N d 2(a) n on i 222n o1.05N d1.05 10 15 cm 31.05 10150.5 10 1520.5 10152n i2son i 25.25 10 28Now3n i 22.8 1019 1.04 1019T300exp1.120.0259 T 30035.25 10 28 2.912 10 38 T300exp 12972.973TBy trial and error, T 536.5K(b) At T 300 K,E c EF kT ln N cn oE c EF 0.0259 ln 2.8 1019 1015T 536.5 K, 0.2652 eVAt536.5kT0.02590.046318 eV3003 / 2N c 2.8 1019 536.53006.696 1019cm 3E c E FN c kT lnn oE c E F6.696 10 19 0.046318 ln10151.050.5124 eVthen E c E F 0.2472 eV(c)Closer to the intrinsic energy level._______________________________________4.51p oE Fi EF kT lnn iAt T 200K, kT 0.017267 eVT 400 K, kT 0.034533 eVT 600 K, kT 0.0518 eV At T 200K,22.8 10191019 200n i 1.04300exp1.120.017267n i 7.638 10 4 cm 3At T 400 K,3n i 2 2.8 1019 1.04 10 19 4003001.12exp0.034533n i 2.381 1012 cm 3At T 600 K,322.8 1019 19 600n i 1.04 10300exp 1.120.0518n i 9.740 1014 cm 3At T 200 K and T 400 K,p o N a 3 1015 cm 3At T 600 K,N a N a2p o n i22 23 15 3 10 15 2 9.740 10 1410 22 23.288 1015cm3Then, T 200K, E Fi E F 0.4212eVT 400K,E Fi EF 0.2465 eVT600K,E Fi EF 0.0630 eV_______________________________________4.52(a)N a N aE Fi EF kT ln 0.0259 ln6n i 1.8 10For N a10 14 cm 3 ,E FiE F0.4619 eVN a 10 15 cm 3,E FiE F0.5215 eV163,N a 10 cmE FiE F0.5811 eVN a 10 17cm 3,E FiE F 0.6408 eV(b)E FEN7.0 1018kT ln0.0259 lnN aN aFor N a10 14 cm 3 ,E F E0.2889 eVN a 10 15 cm 3 ,E FE0.2293 eV163,N a 10 cmE F E0.1697 eVN a 10 17 cm3,E F E 0.1100 eV_______________________________________ 4.53(a) E Fi3 m *p E midgapkT ln4m n *3 0.0259 ln 104 orE Fi E midgap 0.0447 eV(b) Impurity atoms to be added soE midgap EF 0.45 eV(i) p-type, so add acceptor atoms(ii)E Fi EF 0.0447 0.45 0.4947 eVThenp oE FiE Fn i expkT10 5exp 0.49470.0259 or10 13 cm3p o N a1.97_______________________________________4.54n oN d N aN c expE c E FkTsoN d 5 10 15 2.8 10 19 exp0.2150.025951015 6.95 1015orcm 3N d 1.2 1016_______________________________________4.55(a) Silicon(i) E cE F N ckT lnN d0.0259 ln 2.8 10 190.2188 eV6 1015(ii) E cE F0.2188 0.0259 0.1929 eVN dN c expE c E FkT2.8 10 19 exp0.19290.0259N d1.631 1016 cm3N d 6 1015N d1.031 10 16 cm 3Additional donor atoms(b) GaAs(i) E c E F0.0259 ln4.7101710150.15936eV(ii) E cE F0.15936 0.0259 0.13346 eVN d4.7 1017 exp0.133460.02592.718 1015 cm 3N d 1015N d1.718 10 15 cm3Additionaldonor atoms_______________________________________ 4.56(a) E Fi E FN kT lnN a0.0259 ln 1.04 10190.1620 eV2 1016(b) E F E Fi kT ln N c N d0.0259 ln 2.8 1019 0.1876 eV2 10 16(c) For part (a);p o 2 1016 cm 3n i2 1.5 1010 2n op o 2 10161.125 104cm3For part (b):3n o 2 1016 cmn i 2 1.5 1010 2p on o 2 10 161.125 104cm3_______________________________________ 4.57n oE F E Fin i expkT1.8 10 6 exp 0.550.02593.0 1015cm 3Add additional acceptor impuritiesn o N d N a3 10 15 7 10 15 N aN a 4 10 15 cm 3_______________________________________(a) E Fi E F kT lnpon i0.02593 10 150.3161 eVln10 101.5(b) E F E Fin okT lnn i0.02593 10160.3758 eVln10 101.5(c) E F E Fi(d) E Fi E Fp okT lnn i0.0259 375 ln 4 1015300 7.334 10 110.2786 eV(e) E F E Fi kT lnnon i140.0259 450 ln 1.029 10300 1.722 10 130.06945eV_______________________________________4.59(a) E F ENkT lnp o0.0259 ln7.0 10180.2009 eV3 1015(b) E F E 0.0259 l n7.0 10 181.08 10 41.360 eV(c) E F E 0.0259 l n 7.0 10181.8 10 60.7508 eV4.58(d) E F E 0.0259 375300ln 7.0 10 18 375 300 3 / 24 10 150.2526 eV(e) E F E 0.0259 450 300ln 7.0 10 18 450 300 3/ 21.48 10 71.068 eV_______________________________________4.60n-typeE F E Fi kT ln n o n i0.02591.125 10 16ln100.3504 eV1.5 10______________________________________ 4.61N a N a 2 p o 22 2 n i5.08 1015 5 101525 10 15 2n i225.08 10 15 2.5 10 15 22.5 1015 2n i26.6564 10 30 6.25 10 30 n i2n i 2 4.064 10 29n i2 N c N expE gkTkT 0.02593500.030217 eV3003502N c 1.2 10 19 1.633 1019 cm 33003502N 1.8 1019 2.45 10 19 cm 3300Now4.064 10 29 1.633 1019 2.45 1019E gexp0.030217SoE g 0.030217 ln 1.633 10 19 2.45 10 194.064 10 29E g 0.6257 eV_______________________________________4.62(a) Replace Ga atoms Silicon acts as adonorN d0.05 7 1015 3.5 10 14 cm 3Replace As atoms Silicon acts asanacceptorN a 0.95 7 1015 6.65 10 15 cm 3(b) N a N d p-type(c) p o N a N d 6.65 1015 3.5 10146.3 1015cm 3n i 2 1. 810 6 2n o 5.14 10 4 cm 3 p o 6 .3 1015(d) E Fi E F kT ln p o n i0.0259 ln 6.3 10 150.5692 eV1.8 10 6_______________________________________。

半导体物理与器件第四版答案

半导体物理与器件第四版答案半导体物理与器件第四版答案【篇一：半导体物理第五章习题答案】>1. 一个n型半导体样品的额外空穴密度为1013cm-3，已知空穴寿命为100?s，计算空穴的复合率。

解：复合率为单位时间单位体积内因复合而消失的电子-空穴对数，因此1013u1017cm?3?s ?6100?102. 用强光照射n型样品，假定光被均匀吸收，产生额外载流子，产生率为gp，空穴寿命为?，请①写出光照开始阶段额外载流子密度随时间变化所满足的方程；②求出光照下达到稳定状态时的额外载流子密度。

解：⑴光照下，额外载流子密度?n=?p，其值在光照的开始阶段随时间的变化决定于产生和复合两种过程，因此，额外载流子密度随时间变化所满足的方程由产生率gp和复合率u的代数和构成，即 d(?p)?p gp? dt?d(?p)0，于是由上式得⑵稳定时额外载流子密度不再随时间变化，即dtp?p?p0?gp?3. 有一块n型硅样品，额外载流子寿命是1?s，无光照时的电阻率是10??cm。

今用光照射该样品，光被半导体均匀吸收，电子-空穴对的产生率是1022/cm3?s，试计算光照下样品的电阻率，并求电导中少数载流子的贡献占多大比例？解：光照被均匀吸收后产生的稳定额外载流子密度p??n?gp??1022?10?6?1016 cm－3取?n?1350cm2/(v?s)，?p?500cm/(v?s)，则额外载流子对电导率的贡献2pq(?n??p)?1016?1.6?10?19?(1350?500)?2.96 s/cm无光照时?0?10.1s/cm，因而光照下的电导率02.96?0.1?3.06s/cm相应的电阻率 ??110.33??cm 3.06少数载流子对电导的贡献为：?p?pq?p??pq?p?gp?q?p代入数据：?p?(p0??p)q?p??pq?p?1016?1.6?10?19?500?0.8s/cm∴p?00.80.26?26﹪ 3.06即光电导中少数载流子的贡献为26﹪4.一块半导体样品的额外载流子寿命? =10?s，今用光照在其中产生非平衡载流子，问光照突然停止后的20?s时刻其额外载流子密度衰减到原来的百分之几？解：已知光照停止后额外载流子密度的衰减规律为p(t)??p0e?因此光照停止后任意时刻额外载流子密度与光照停止时的初始密度之比即为t??p(t)e? ?p0t当t?20?s?2?10?5s时20??p(20)e10?e?2?0.135?13.5﹪ ?p05. 光照在掺杂浓度为1016cm-3的n型硅中产生的额外载流子密度为?n=?p= 1016cm-3。

半导体物理与器件第四版课后习题答案1

______________________________________________________________________________________Chapter 1Problem Solutions1.1 (a)fcc: 8 corner atoms 18/1atom6 face atoms32/1atomsTotal of 4 atoms per unit cell (b)bcc: 8 corner atoms 18/1atom1 enclosed atom=1 atom Total of 2 atoms per unit cell(c)Diamond: 8 corner atoms 18/1atom6 faceatoms 32/1atoms4 enclosedatoms= 4 atomsTotal of 8 atoms per unit cell_______________________________________ 1.2 (a)Simple cubic lattice: r a 2Unit cell vol33382rra1 atom per cell, so atom vol 3413r ThenRatio%4.52%10083433rr(b)Face-centered cubic latticerd aa rd22224Unit cell vol 33321622rr a4 atoms per cell, so atom vol3443r ThenRatio%74%10021634433rr (c)Body-centered cubic latticeraa rd3434Unit cell vol 3334ra2 atoms per cell, so atom vol 3423r ThenRatio%68%1003434233r r (d)Diamond lattice Body diagonal raa rd3838Unit cell vol3338r a8 atoms per cell, so atom vol 3483r ThenRatio%34%1003834833rr _______________________________________1.3(a)oA a43.5; From Problem 1.2d,ra38Then oAa r176.18343.583Center of one silicon atom to center ofnearest neighboroAr 35.22______________________________________________________________________________________ (b)Number density22381051043.58cm 3(c)Mass density23221002.609.28105..AN W t At N 33.2grams/cm3_______________________________________1.4(a)4 Ga atoms per unit cell Number density381065.54Density of Ga atoms 221022.2cm34 As atoms per unit cell Density of As atoms 221022.2cm3(b)8 Ge atoms per unit cell Number density381065.58Density of Ge atoms221044.4cm3_______________________________________ 1.5From Figure 1.15 (a)aa d4330.0232oAd 447.265.54330.0(b)aa d7071.022oAd 995.365.57071.0_______________________________________1.674.5423232222sin a a 5.109_______________________________________ 1.7(a) Simple cubic: oAr a 9.32(b)fcc:oAr a515.524(c) bcc:oA r a 503.434(d) diamond:oAra007.9342_______________________________________ 1.8 (a)Br 2035.122035.12oBAr 4287.0(b)oAa 07.2035.12(c)A-atoms: # of atoms1818Density381007.21231013.1cm3B-atoms: # of atoms3216Density381007.23231038.3cm3_______________________________________ 1.9(a)oAr a 5.42# of atoms1818Number density38105.412210097.1cm3______________________________________________________________________________________Mass density AN W t At N ..23221002.65.12100974.1228.0gm/cm3(b)oAr a196.534# of atoms 21818Number density3810196.5222104257.1cm3Mass density23221002.65.12104257.1296.0gm/cm3_______________________________________ 1.10From Problem 1.2, percent volume of fcc atoms is 74%; Therefore after coffee is ground,Volume = 0.74 cm3_______________________________________1.11(b)oAa 8.20.18.1(c)Na: Density38108.22/1221028.2cm3Cl: Density221028.2cm3(d)Na: At. Wt. = 22.99 Cl: At. Wt. = 35.45 So, mass per unit cell23231085.41002.645.352199.2221Then mass density21.2108.21085.43823grams/cm3_______________________________________ 1.12(a)oAa 88.122.223Then oA a 62.4Density of A:22381001.11062.41cm3Density of B:22381001.11062.41cm3(b)Same as (a) (c)Same material_______________________________________ 1.13oAa619.438.122.22(a) For 1.12(a), A-atomsSurface density28210619.411a1410687.4cm2For 1.12(b), B-atoms: oAa 619.4Surface density14210687.41acm2For 1.12(a) and (b), Same material(b) For 1.12(a), A-atoms;oAa 619.4Surface density212a1410315.3cm2B-atoms;Surface density______________________________________________________________________________________14210315.321a cm 2For 1.12(b), A-atoms;oAa 619.4Surface density212a1410315.3cm2B-atoms;Surface density14210315.321acm2For 1.12(a) and (b), Same material_______________________________________ 1.14 (a)Vol. Density31oaSurface Density212oa(b)Same as (a)_______________________________________ 1.15 (i)(110) plane(see Figure 1.10(b))(ii) (111) plane(see Figure 1.10(c))(iii) (220) plane,1,1,21,21Same as (110) plane and [110]direction(iv) (321) plane6,3,211,21,31Intercepts of plane at6,3,2sq p [321] direction is perpendicular to(321) plane_______________________________________1.16(a)31311,31,11(b)12141,21,41_______________________________________ 1.17Intercepts: 2, 4, 331,41,21(634) plane_______________________________________ 1.18(a)oAa d 28.5(b)oAa d734.322(c)oAa d048.333_______________________________________ 1.19(a) Simple cubic(i) (100) plane:Surface density2821073.411a141047.4cm 2(ii) (110) plane:Surface density212a141016.3cm 2(iii) (111) plane: Area of planebh21where oAa b 689.62Now2222243222a a a hSooAh793.573.426______________________________________________________________________________________Area of plane881079304.51068923.62116103755.19cm 2Surface density16103755.19613141058.2cm2(b) bcc(i) (100) plane:Surface density 1421047.41acm2(ii) (110) plane: Surface density222a141032.6cm 2(iii) (111) plane:Surface density16103755.19613141058.2cm2(c) fcc(i) (100) plane:Surface density 1421094.82acm2(ii) (110) plane: Surface density222a141032.6cm 2(iii) (111) plane:Surface density16103755.19213613151003.1cm2_______________________________________ 1.20 (a)(100) plane: - similar to a fcc:Surface density281043.52141078.6cm 2(b)(110) plane:Surface density281043.524141059.9cm2(c)(111) plane: Surface density281043.5232141083.7cm2_______________________________________1.21oAr a703.6237.2424(a)#/cm338310703.64216818a2210328.1cm3(b)#/cm222124142a210703.62281410148.3cm2(c)oA a d74.422703.622(d)# of atoms2213613Area of plane: (see Problem 1.19)oAa b4786.92oAa h2099.826Area88102099.8104786.92121bh______________________________________________________________________________________15108909.3cm2#/cm215108909.32=141014.5cm2oAa d87.333703.633_______________________________________ 1.22Density of silicon atoms 22105cm3and4 valence electrons per atom, soDensity of valence electrons 23102cm3_______________________________________ 1.23Density of GaAs atoms22381044.41065.58cm3An average of 4 valence electrons peratom,SoDensity of valence electrons231077.1cm3_______________________________________ 1.24 (a)%10%10010510532217(b)%104%10010510262215_______________________________________ 1.25 (a)Fraction by weight7221610542.106.2810582.10102(b)Fraction by weight5221810208.206.2810598.3010_______________________________________ 1.26Volume density 1631021dcm3So610684.3dcmoAd 4.368We haveoo Aa 43.5Then85.6743.54.368oa d _______________________________________ 1.27Volume density 1531041dcm 3So61030.6dcmoAd630We have oo Aa 43.5Then11643.5630oa d _______________________________________。

半导体物理与器件第四版课后答案第六章

E 3.15 10 19 J; energy of one
photon Now 1 W = 1 J/s 3.17 1018 photons/s Volume = (1)(0.1) = 0.1 cm 3 Then 3.17 1018 g 0.1

2
1.62 10 4 cm 3
10149.25.124 1013 1.124 1013 1015.124 1013 49.21.124 1013
54.2 cm 2 /s
and
kT (b) D D n n 0.0259 1300 e
We find n nD p p pD n 2 n

n p p n n

n n p p g R

Semiconductor Physics and Devices: Basic Principles, 4th edition Chapter 6 By D. A. Neamen Problem Solutions ______________________________________________________________________________________ 3.214 10 4 7 1015 n pt 10 7 nn p p t so pt 2.18 10 4 s Divide by n n p p , then _______________________________________ n nD p p pD n 2 n nn p p 6.10 For Ge: n i 2.4 1013 cm 3

半导体物理与器件第四版课后习题答案(完整教资)

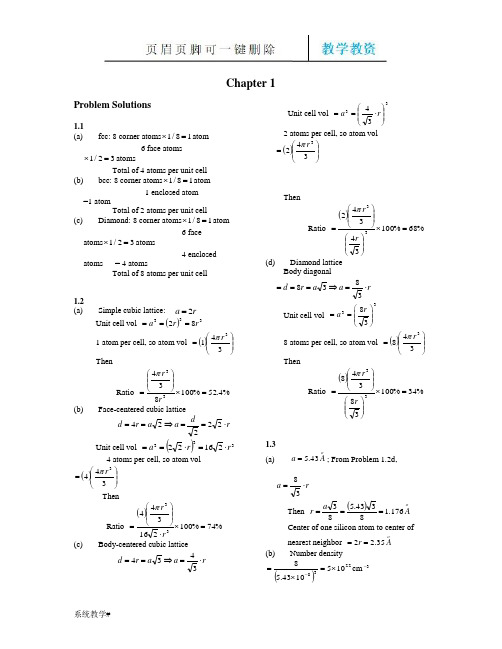
Chapter 1Problem Solutions1.1 (a) fcc: 8 corner atoms 18/1=⨯atom 6 face atoms 32/1=⨯atomsTotal of 4 atoms per unit cell (b) bcc: 8 corner atoms 18/1=⨯atom1 enclosed atom =1 atomTotal of 2 atoms per unit cell (c) Diamond: 8 corner atoms 18/1=⨯atom 6 face atoms 32/1=⨯atoms4 enclosed atoms = 4 atomsTotal of 8 atoms per unit cell_______________________________________ 1.2 (a) Simple cubic lattice: r a 2=Unit cell vol ()33382r r a === 1 atom per cell, so atom vol ()⎪⎪⎭⎫⎝⎛=3413r π ThenRatio %4.52%10083433=⨯⎪⎪⎭⎫ ⎝⎛=rr π (b) Face-centered cubic latticer da a r d ⋅==⇒==22224Unit cell vol ()33321622r r a ⋅=⋅==4 atoms per cell, so atom vol()⎪⎪⎭⎫⎝⎛=3443r π ThenRatio ()%74%10021634433=⨯⋅⎪⎪⎭⎫⎝⎛=r r π (c) Body-centered cubic latticer a a r d ⋅=⇒==3434 Unit cell vol 3334⎪⎪⎭⎫⎝⎛⋅==r a 2 atoms per cell, so atom vol()⎪⎪⎭⎫⎝⎛=3423r πThenRatio ()%68%1003434233=⨯⎪⎪⎭⎫ ⎝⎛⎪⎪⎭⎫⎝⎛=r r π (d) Diamond lattice Body diagonalr a a r d ⋅=⇒===3838Unit cell vol 3338⎪⎪⎭⎫⎝⎛==r a 8 atoms per cell, so atom vol ()⎪⎪⎭⎫⎝⎛=3483r π ThenRatio ()%34%1003834833=⨯⎪⎪⎭⎫ ⎝⎛⎪⎪⎭⎫⎝⎛=r r π _______________________________________ 1.3(a)oA a 43.5=; From Problem 1.2d,r a ⋅=38Then ()o A a r 176.18343.583===Center of one silicon atom to center ofnearest neighbor oA r 35.22== (b) Number density()22381051043.58⨯=⨯=-cm 3-(c)Mass density()()()23221002.609.28105..⨯⨯===A N W t At N ρ 33.2=⇒ρ grams/cm 3_______________________________________ 1.4 (a) 4 Ga atoms per unit cellNumber density ()381065.54-⨯=⇒Density of Ga atoms221022.2⨯=cm 3-4 As atoms per unit cell ⇒Density of As atoms 221022.2⨯=cm 3- (b) 8 Ge atoms per unit cellNumber density ()381065.58-⨯=⇒Density of Ge atoms 221044.4⨯=cm 3-_______________________________________ 1.5From Figure 1.15(a)()a a d 4330.0232=⎪⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛= =()()oA d 447.265.54330.0=⇒ (b)()a a d 7071.022=⎪⎭⎫⎝⎛=()()oA d 995.365.57071.0=⇒= _______________________________________ 1.6︒=⇒==⎪⎭⎫ ⎝⎛74.5423232222sin θθa a︒=⇒5.109θ_______________________________________ 1.7(a) Simple cubic: oA r a 9.32== (b) fcc: oA r a 515.524==(c) bcc: oA ra 503.434==(d) diamond: ()oA r a 007.9342==_______________________________________ 1.8(a)()()B r 2035.122035.12+= oB A r 4287.0= (b) ()oA a 07.2035.12==(c)A-atoms: # of atoms 1818=⨯= Density ()381007.21-⨯=231013.1⨯=cm 3-B-atoms: # of atoms 3216=⨯=Density ()381007.23-⨯=231038.3⨯= cm 3- _______________________________________ 1.9 (a)oA r a 5.42==# of atoms 1818=⨯= Number density ()38105.41-⨯=2210097.1⨯=cm 3-Mass density ()AN W t At N ..==ρ ()()23221002.65.12100974.1⨯⨯==228.0gm/cm 3(b)o A ra 196.534==# of atoms 21818=+⨯Number density ()3810196.52-⨯=22104257.1⨯=cm 3-Mass density ()()23221002.65.12104257.1⨯⨯==ρ296.0=gm/cm 3_______________________________________ 1.10From Problem 1.2, percent volume of fcc atoms is 74%; Therefore after coffee is ground,Volume = 0.74 cm 3_______________________________________ 1.11(b)oA a 8.20.18.1=+= (c)Na: Density ()()38108.22/1-⨯=221028.2⨯=cm 3-Cl: Density 221028.2⨯=cm 3- (d) Na: At. Wt. = 22.99Cl: At. Wt. = 35.45So, mass per unit cell ()()23231085.41002.645.352199.2221-⨯=⨯⎪⎭⎫⎝⎛+⎪⎭⎫ ⎝⎛= Then mass density()21.2108.21085.43823=⨯⨯=--ρ grams/cm 3_______________________________________ 1.12 (a)()()oA a 88.122.223=+=Then oA a 62.4= Density of A:()22381001.11062.41⨯=⨯=-cm 3-Density of B: ()22381001.11062.41⨯=⨯=-cm 3-(b) Same as (a) (c) Same material_______________________________________ 1.13()()o A a 619.438.122.22=+=(a) For 1.12(a), A-atoms Surface density ()28210619.411-⨯==a 1410687.4⨯=cm 2-For 1.12(b), B-atoms: oA a 619.4= Surface density 14210687.41⨯==acm 2- For 1.12(a) and (b), Same material(b) For 1.12(a), A-atoms; o A a 619.4= Surface density212a =1410315.3⨯=cm 2-B-atoms;Surface density14210315.321⨯==a cm 2- For 1.12(b), A-atoms; o A a 619.4= Surface density212a =1410315.3⨯=cm 2-B-atoms;Surface density14210315.321⨯==a cm 2- For 1.12(a) and (b), Same material_______________________________________ 1.14(a) Vol. Density 31oa =Surface Density 212oa=(b) Same as (a)_______________________________________ 1.15 (i) (110) plane(see Figure 1.10(b))(ii) (111) plane(see Figure 1.10(c))(iii) (220) plane ⇒()0,1,1,21,21⇒⎪⎭⎫⎝⎛∞Same as (110) plane and [110] direction(iv) (321) plane ()6,3,211,21,31⇒⎪⎭⎫⎝⎛⇒Intercepts of plane at 6,3,2===s q p[321] direction is perpendicular to (321) plane_______________________________________ 1.16(a)()31311,31,11⇒⎪⎭⎫⎝⎛(b)()12141,21,41⇒⎪⎭⎫⎝⎛_______________________________________ 1.17Intercepts: 2, 4, 3 ⇒⎪⎭⎫⎝⎛⇒31,41,21(634) plane_______________________________________ 1.18(a) oA a d 28.5==(b) o A a d 734.322==(c) o A a d 048.333==_______________________________________ 1.19 (a) Simple cubic(i) (100) plane:Surface density ()2821073.411-⨯==a141047.4⨯=cm 2- (ii) (110) plane:Surface density 212a =141016.3⨯=cm 2- (iii) (111) plane:Area of plane bh 21=where oA a b 689.62== Now ()()2222243222a a a h =⎪⎪⎭⎫⎝⎛-= So ()o A h 793.573.426==Area of plane ()()881079304.51068923.621--⨯⨯= 16103755.19-⨯=cm 2Surface density 16103755.19613-⨯⨯=141058.2⨯=cm 2- (b) bcc(i) (100) plane:Surface density 1421047.41⨯==a cm 2- (ii) (110) plane:Surface density 222a =141032.6⨯=cm 2- (iii) (111) plane:Surface density 16103755.19613-⨯⨯=141058.2⨯=cm 2- (c) fcc(i) (100) plane:Surface density 1421094.82⨯==acm 2-(ii) (110) plane:Surface density 222a =141032.6⨯=cm 2- (iii) (111) plane:Surface density 16103755.19213613-⨯⨯+⨯=151003.1⨯=cm 2-_______________________________________ 1.20 (a) (100) plane: - similar to a fcc:Surface density ()281043.52-⨯=141078.6⨯=cm 2- (b) (110) plane:Surface density ()281043.524-⨯=141059.9⨯=cm 2- (c) (111) plane:Surface density ()()281043.5232-⨯= 141083.7⨯=cm 2-_______________________________________ 1.21()o A r a 703.6237.2424===(a) #/cm 3()38310703.64216818-⨯=⨯+⨯=a 2210328.1⨯=cm 3-(b) #/cm 222124142a ⨯+⨯= ()210703.6228-⨯=1410148.3⨯=cm 2- (c) ()o A a d 74.422703.622===(d)# of atoms 2213613=⨯+⨯=Area of plane: (see Problem 1.19)oA a b 4786.92==o A ah 2099.826==Area ()()88102099.8104786.92121--⨯⨯==bh 15108909.3-⨯=cm 2#/cm 215108909.32-⨯= =141014.5⨯ cm 2-()o A a d 87.333703.633===_______________________________________ 1.22Density of silicon atoms 22105⨯=cm 3- and4 valence electrons per atom, so Density of valence electrons 23102⨯=cm 3-_______________________________________ 1.23Density of GaAs atoms()22381044.41065.58⨯=⨯=-cm 3- An average of 4 valence electrons per atom,SoDensity of valence electrons231077.1⨯=cm 3-_______________________________________ 1.24(a) %10%10010510532217-=⨯⨯⨯ (b) %104%10010510262215-⨯=⨯⨯⨯ _______________________________________ 1.25 (a) Fraction by weight()()()()7221610542.106.2810582.10102-⨯=⨯⨯≅ (b) Fraction by weight()()()()5221810208.206.2810598.3010-⨯=⨯≅ _______________________________________ 1.26Volume density 1631021⨯==dcm 3-So 610684.3-⨯=d cm oA d 4.368=⇒ We have oo A a 43.5=Then85.6743.54.368==o a d _______________________________________ 1.27Volume density 1531041⨯==dcm 3-So 61030.6-⨯=d cm oA d 630=⇒ We have oo A a 43.5= Then11643.5630==o a d _______________________________________。

《半导体物理与器件》第四版答案第十章

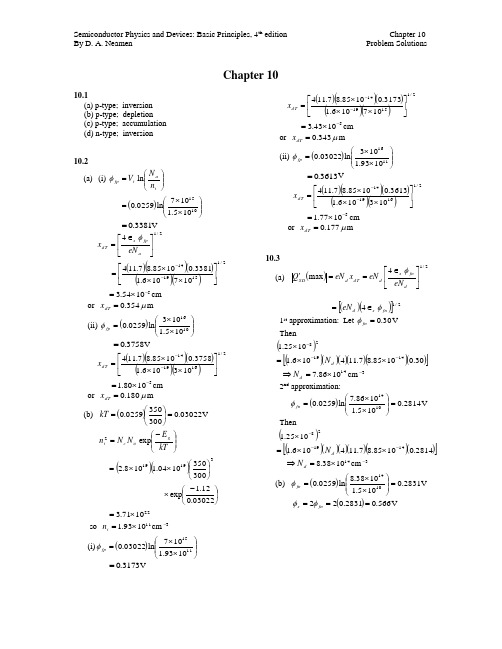
Chapter 1010.1(a) p-type; inversion (b) p-type; depletion (c) p-type; accumulation (d) n-type; inversion_______________________________________ 10.2(a) (i) ⎪⎪⎭⎫⎝⎛=i a t fp n N V ln φ ()⎪⎪⎭⎫ ⎝⎛⨯⨯=1015105.1107ln 0259.0 3381.0=V 2/14⎥⎦⎤⎢⎣⎡∈=a fp s dTeN x φ()()()()()2/1151914107106.13381.01085.87.114⎥⎦⎤⎢⎣⎡⨯⨯⨯=--51054.3-⨯=cm or μ354.0=dT x m(ii) ()⎪⎪⎭⎫⎝⎛⨯⨯=1016105.1103ln 0259.0fp φ3758.0=V ()()()()()2/1161914103106.13758.01085.87.114⎥⎦⎤⎢⎣⎡⨯⨯⨯=--dTx51080.1-⨯=cmor μ180.0=dT x m(b) ()03022.03003500259.0=⎪⎭⎫⎝⎛=kT V⎪⎪⎭⎫⎝⎛-=kT E N N n g c i exp 2υ ()()319193003501004.1108.2⎪⎭⎫⎝⎛⨯⨯=⎪⎭⎫⎝⎛-⨯03022.012.1exp221071.3⨯=so 111093.1⨯=i n cm 3-(i)()⎪⎪⎭⎫⎝⎛⨯⨯=11151093.1107ln 03022.0fp φ3173.0=V()()()()()2/1151914107106.13173.01085.87.114⎥⎦⎤⎢⎣⎡⨯⨯⨯=--dT x51043.3-⨯=cm or μ343.0=dT x m(ii) ()⎪⎪⎭⎫⎝⎛⨯⨯=11161093.1103ln 03022.0fp φ3613.0=V()()()()()2/1161914103106.13613.01085.87.114⎥⎦⎤⎢⎣⎡⨯⨯⨯=--dT x51077.1-⨯=cm or μ177.0=dT x m_______________________________________ 10.3(a) ()2/14m ax ⎥⎦⎤⎢⎣⎡∈=='d fn s d dT d SDeN eN x eN Q φ()()[]2/14fns d eN φ∈=1st approximation: Let 30.0=fn φV Then()281025.1-⨯()()()()()()[]30.01085.87.114106.11419--⨯⨯=dN 141086.7⨯=⇒d N cm 3-2nd approximation:()2814.0105.11086.7ln 0259.01014=⎪⎪⎭⎫⎝⎛⨯⨯=fn φV Then ()281025.1-⨯()()()()()()[]2814.01085.87.114106.11419--⨯⨯=d N 141038.8⨯=⇒d N cm 3-(b) ()2831.0105.11038.8ln 0259.01014=⎪⎪⎭⎫⎝⎛⨯⨯=fn φV()566.02831.022===fn s φφV _______________________________________10.4 p-type silicon (a) Aluminum gate ⎥⎥⎦⎤⎢⎢⎣⎡⎪⎪⎭⎫ ⎝⎛++'-'=fp g m ms e E φχφφ2 We have ⎪⎪⎭⎫ ⎝⎛=i a t fp n N V ln φ ()334.0105.1106ln 0259.01015=⎪⎪⎭⎫ ⎝⎛⨯⨯=V Then()[]334.056.025.320.3++-=ms φ or 944.0-=ms φV (b) +n polysilicon gate ⎪⎪⎭⎫⎝⎛+-=fp g ms e E φφ2()334.056.0+-= or 894.0-=ms φV (c) +p polysilicon gate ()334.056.02-=⎪⎪⎭⎫⎝⎛-=fp g ms e E φφ or226.0+=ms φV_______________________________________ 10.5()3832.0105.1104ln 0259.01016=⎪⎪⎭⎫ ⎝⎛⨯⨯=fp φV ⎪⎪⎭⎫⎝⎛++'-'=fp g m ms e E φχφφ2 ()3832.056.025.320.3++-= 9932.0-=ms φV _______________________________________10.6 (a) 17102⨯≅d N cm 3- (b) Not possible - ms φ is always positive.(c) 15102⨯≅d N cm 3-_______________________________________10.7 From Problem 10.5, 9932.0-=ms φV ox ssms FB C Q V '-=φ (a) ()()814102001085.89.3--⨯⨯=∈=ox ox ox t C 710726.1-⨯=F/cm 2()()7191010726.1106.11059932.0--⨯⨯⨯--=FB V 040.1-=V (b) ()()81410801085.89.3--⨯⨯=ox C 710314.4-⨯=F/cm 2 ()()7191010314.4106.11059932.0--⨯⨯⨯--=FB V012.1-=V _______________________________________10.8 (a) 42.0-≅ms φV 42.0-==ms FB V φV(b) ()()781410726.1102001085.89.3---⨯=⨯⨯=ox C F/cm 2 (i)()()7191010726.1106.1104--⨯⨯⨯-='-=∆ox ss FB C Q V 0371.0-=V (ii)()()7191110726.1106.110--⨯⨯-=∆FB V 0927.0-=V(c) 42.0-==ms FB V φV ()()781410876.2101201085.89.3---⨯=⨯⨯=ox C F/cm 2 (i)()()7191010876.2106.1104--⨯⨯⨯-=∆FB V 0223.0-=V (ii)()()7191110876.2106.110--⨯⨯-=∆FB V0556.0-=V _______________________________________10.9 ⎪⎪⎭⎫ ⎝⎛++'-'=fp g mms e E φχφφ2 where()365.0105.1102ln 0259.01016=⎪⎪⎭⎫ ⎝⎛⨯⨯=fp φV Then ()365.056.025.320.3++-=ms φor975.0-=ms φVNowox ss ms FB C Q V '-=φor ()ox FB ms ss C V Q -='φ We have()()814104501085.89.3--⨯⨯=∈=ox ox ox t C or 81067.7-⨯=ox C F/cm 2 So now ()[]()81067.71975.0-⨯⋅---='ssQ 91092.1-⨯=C/cm 2or10102.1⨯='e Q ss cm 2- _______________________________________10.10 ()3653.0105.1102ln 0259.01016=⎪⎪⎭⎫ ⎝⎛⨯⨯=fp φV ()()()()()2/1161914102106.13653.01085.87.114⎥⎦⎤⎢⎣⎡⨯⨯⨯=--dT x510174.2-⨯=cm()dT a SDx eN Q ='m ax ()()()5161910174.2102106.1--⨯⨯⨯=810958.6-⨯=C/cm 2()()781410301.2101501085.89.3---⨯=⨯⨯=ox C F/cm 2()fp ms ox ss SDTN C Q Q V φφ2max ++'-'= ()()71910810301.2106.110710958.6---⨯⨯⨯-⨯= ()3653.02++ms φ ms φ+=9843.0(a) n + poly gate on p-type: 12.1-≅ms φV 136.012.19843.0-=-=TN V V(b) p + poly gate on p-type: 28.0+≅ms φV 26.128.09843.0+=+=TN V V (c) Al gate on p-type: 95.0-≅ms φV0343.095.09843.0+=-=TN V V_______________________________________10.11 ()3161.0105.1103ln 0259.01015=⎪⎪⎭⎫ ⎝⎛⨯⨯=fn φV ()()()()()2/1151914103106.13161.01085.87.114⎥⎦⎤⎢⎣⎡⨯⨯⨯=--dT x 510223.5-⨯=cm ()dT d SDx eN Q ='m ax ()()()5151910223.5103106.1--⨯⨯⨯= 810507.2-⨯=C/cm 2 ()()781410301.2101501085.89.3---⨯=⨯⨯=ox C F/cm 2 ()fn ms ox ss SDTP C Q Q V φφ2m ax -+⎥⎥⎦⎤⎢⎢⎣⎡'+'-= ()()⎥⎦⎤⎢⎣⎡⨯⨯⨯+⨯-=---71019810301.2107106.110507.2 ()3161.02-+ms φ ms TP V φ+-=7898.0(a) n + poly gate on n-type: 41.0-≅ms φV 20.141.07898.0-=--=TP V V(b) p + poly gate on n-type: 0.1+≅ms φV 210.00.17898.0+=+-=TP V V (c) Al gate on n-type: 29.0-≅ms φV 08.129.07898.0-=--=TP V V _______________________________________10.12()3294.0105.1105ln 0259.01015=⎪⎪⎭⎫ ⎝⎛⨯⨯=fp φV The surface potential is ()659.03294.022===fp s φφV We have 90.0-='-=oxssms FB C Q V φV Now()FB s oxSDT V C Q V ++'=φmaxWe obtain 2/14⎥⎦⎤⎢⎣⎡∈=a fp s dTeN x φ()()()()()2/1151914105106.13294.01085.87.114⎥⎦⎤⎢⎣⎡⨯⨯⨯=-- or410413.0-⨯=dT x cm Then()()()()4151910413.0105106.1m ax --⨯⨯⨯='SDQ or()810304.3m ax -⨯='SDQ C/cm 2 We also find()()814104001085.89.3--⨯⨯=∈=ox ox ox t C or810629.8-⨯=ox C F/cm 2 Then90.0659.010629.810304.388-+⨯⨯=--T Vor142.0+=T V V_______________________________________10.13()()814102201085.89.3--⨯⨯=∈=ox ox ox t C 710569.1-⨯=F/cm 2()()1019104106.1⨯⨯='-ssQ 9104.6-⨯=C/cm 2By trial and error, let 16104⨯=a N cm 3-.Now ()⎪⎪⎭⎫⎝⎛⨯⨯=1016105.1104ln 0259.0fp φ3832.0=V()()()()()2/1161914104106.13832.01085.87.114⎥⎦⎤⎢⎣⎡⨯⨯⨯=--dT x 510575.1-⨯=cm ()m ax SDQ ' ()()()5161910575.1104106.1--⨯⨯⨯= 710008.1-⨯=C/cm 294.0-≅ms φV Then()fp ms oxss SDTN C Q Q V φφ2max ++'-'=79710569.1104.610008.1---⨯⨯-⨯=()3832.0294.0+- Then 428.0=TN V V 45.0≅V_______________________________________10.14()()814101801085.89.3--⨯⨯=∈=ox ox ox t C 7109175.1-⨯=F/cm 3- ()()1019104106.1⨯⨯='-ssQ 9104.6-⨯=C/cm 2By trial and error, let 16105⨯=d N cm 3- Now()⎪⎪⎭⎫⎝⎛⨯⨯=1016105.1105ln 0259.0fn φ3890.0=V()()()()()2/1161914105106.13890.01085.87.114⎥⎦⎤⎢⎣⎡⨯⨯⨯=--dT x510419.1-⨯=cm()m ax SDQ ' ()()()5161910419.1105106.1--⨯⨯⨯= 710135.1-⨯=C/cm 3-10.1+≅ms φV Then()()fn ms ox ss SDTP C Q Q V φφ2max -+'+'-= ()797109175.1104.610135.1---⨯⨯+⨯-= ()3890.0210.1-+Then 303.0-=TP V V, which is within thespecified value. _______________________________________ 10.15 We have 710569.1-⨯=ox C F/cm 2 9104.6-⨯='ssQ C/cm 2 By trial and error, let 14105⨯=d N cm 3-Now()⎪⎪⎭⎫⎝⎛⨯⨯=1014105.1105ln 0259.0fn φ 2697.0=V()()()()()2/1141914105106.12697.01085.87.114⎥⎦⎤⎢⎣⎡⨯⨯⨯=--dT x 410182.1-⨯=cm ()m ax SDQ ' ()()()4141910182.1105106.1--⨯⨯⨯= 910456.9-⨯=C/cm 233.0-≅ms φVThen ()()fn ms oxss SDTP C Q Q V φφ2max -+'+'-= ⎪⎪⎭⎫⎝⎛⨯⨯+⨯-=---79910569.1104.610456.9 ()2697.0233.0--970.0=V Then 970.0-=TP V V 975.0-≅ V which meets the specification._______________________________________ 10.16(a) 03.1-≅ms φV()()814101801085.89.3--⨯⨯=ox C 7109175.1-⨯=F/cm 2Now oxss ms FB C Q V '-=φ()()71019109175.1106106.103.1--⨯⨯⨯--= 08.1-=FB V V(b) ()⎪⎪⎭⎫ ⎝⎛⨯=1015105.110ln 0259.0fp φ 2877.0=V ()()()()()2/115191410106.12877.01085.87.114⎥⎦⎤⎢⎣⎡⨯⨯=--dT x 510630.8-⨯=cm()m ax SDQ ' ()()()5151910630.810106.1--⨯⨯= 810381.1-⨯=C/cm 2 Now ()fp FB oxSDTN V C Q V φ2max ++'=()2877.0208.1109175.110381.178+-⨯⨯=-- or 433.0-=TN V V_______________________________________10.17 (a) We have n-type material under the gate, so2/14⎥⎦⎤⎢⎣⎡∈==d fn s C dT eN t x φ where()288.0105.110ln 0259.01015=⎪⎪⎭⎫ ⎝⎛⨯=fn φVThen()()()()()2/115191410106.1288.01085.87.114⎥⎦⎤⎢⎣⎡⨯⨯=--dT x or 410863.0-⨯==C dT t x cm μ863.0=m (b)()()fn ms ox ox ss SD T t Q Q V φφ2max -+⎪⎪⎭⎫⎝⎛∈'+'-= For an +n polysilicon gate, ()288.056.02--=⎪⎪⎭⎫ ⎝⎛--=fn g ms e E φφ or272.0-=ms φV Now ()()()()4151910863.010106.1m ax --⨯⨯='SD Q or ()81038.1m ax -⨯='SDQ C/cm 2 We have()()91019106.110106.1--⨯=⨯='ssQ C/cm 2 We now find ()()()()81498105001085.89.3106.11038.1----⨯⨯⨯+⨯-=T V ()288.02272.0--or 07.1-=T V V _______________________________________ 10.18 (b) ⎪⎪⎭⎫⎝⎛++'-'=fp g m ms e E φχφφ2 where 20.0-='-'χφm V and()3473.0105.110ln 0259.01016=⎪⎪⎭⎫⎝⎛⨯=fp φV Then()3473.056.020.0+--=ms φ or 107.1-=ms φV (c) For 0='ss Q ()fp ms ox ox SDTN t Q V φφ2max ++⎪⎪⎭⎫⎝⎛∈'= We find()()()()()2/116191410106.13473.01085.87.114⎥⎦⎤⎢⎣⎡⨯⨯=--dT xor 41030.0-⨯=dT x cm μ30.0=m Now()()()()416191030.010106.1m ax --⨯⨯='SDQ or ()810797.4m ax -⨯='SDQ C/cm 2Then()()()()14881085.89.31030010797.4---⨯⨯⨯=T V()3473.02107.1+- or00455.0+=T V V 0≅V _______________________________________ 10.19Plot _______________________________________ 10.20 Plot_______________________________________ 10.21 Plot _______________________________________10.22 Plot_______________________________________10.23 (a) For 1=f Hz (low freq), ()()814101201085.89.3--⨯⨯=∈=ox ox ox t C 710876.2-⨯=F/cm 2a st s ox ox oxFB eNV t C ∈⎪⎪⎭⎫ ⎝⎛∈∈+∈=' ()()()()()()()16191481410106.11085.87.110259.07.119.3101201085.89.3----⨯⨯⎪⎭⎫ ⎝⎛+⨯⨯= 710346.1-⨯='FB C F/cm 2 dTs ox ox oxx t C ⋅⎪⎪⎭⎫ ⎝⎛∈∈+∈='minNow ()3473.0105.110ln 0259.01016=⎪⎪⎭⎫ ⎝⎛⨯=fp φV ()()()()()2/116191410106.13473.01085.87.114⎥⎦⎤⎢⎣⎡⨯⨯=--dTx51000.3-⨯=cmThen ()()()5814min 1000.37.119.3101201085.89.3---⨯⎪⎭⎫ ⎝⎛+⨯⨯='C 810083.3-⨯=F/cm 2 C '(inv)710876.2-⨯==ox C F/cm 2 (b) 1=f MHz (high freq), 710876.2-⨯=ox C F/cm 2 (unchanged) 710346.1-⨯='FBC F/cm 2 (unchanged) 8min10083.3-⨯='C F/cm 2 (unchanged) C '(inv)8min10083.3-⨯='=C F/cm 2 (c) 10.1-≅==ms FB V φV()fp FB oxSDTN V C Q V φ2max ++'=Now()dT a SDx eN Q ='m ax ()()()516191000.310106.1--⨯⨯=81080.4-⨯=C/cm 2 ()3473.0210.110876.21080.478+-⨯⨯=--TN V 2385.0-=TN V V_______________________________________10.24(a) 1=f Hz (low freq), ()()814101201085.89.3--⨯⨯=∈=ox ox oxt C 710876.2-⨯=F/cm 2a st s ox ox oxFB eNV t C ∈⎪⎪⎭⎫ ⎝⎛∈∈+∈='()()()()()()()141914814105106.11085.87.110259.07.119.3101201085.89.3⨯⨯⨯⎪⎭⎫ ⎝⎛+⨯⨯=---- 810726.4-⨯='FBC F/cm 2 dTs ox ox oxx t C ⋅⎪⎪⎭⎫⎝⎛∈∈+∈='minNow()2697.0105.1105ln 0259.01014=⎪⎪⎭⎫⎝⎛⨯⨯=fn φV()()()()()2/1141914105106.12697.01085.87.114⎥⎦⎤⎢⎣⎡⨯⨯⨯=--dT x 410182.1-⨯=cmThen()()()4814min 10182.17.119.3101201085.89.3---⨯⎪⎭⎫ ⎝⎛+⨯⨯='C 910504.8-⨯=F/cm 2C '(inv)710876.2-⨯==ox C F/cm 2(b) 1=f MHz (high freq),710876.2-⨯=ox C F/cm 2 (unchanged)810726.4-⨯='FBC F/cm 2 (unchanged) 9min10504.8-⨯='C F/cm 2 (unchanged) C '(inv)9min10504.8-⨯='=C F/cm 2 (c) 95.0≅=ms FB V φV()fn FB oxSDTP V C Q V φ2max -+'-=Now()dT d SDx eN Q ='m ax ()()()4141910182.1105106.1--⨯⨯⨯= 910456.9-⨯=C/cm 2Then()2697.0295.010876.210456.979-+⨯⨯-=--TP V378.0+=TP V V_______________________________________10.25The amount of fixed oxide charge at x is ()x x ∆ρ C/cm 2By lever action, the effect of this oxide charge on the flatband voltage is()x x t x C V ox ox FB ∆⎪⎪⎭⎫⎝⎛-=∆ρ1 If we add the effect at each point, we must integrate so that ()dx t x x C V oxt oxoxFB⎰-=∆01ρ _______________________________________10.26 (a) We have ρx Q t SS ()='∆ Then∆V C x x t dx FB ox ox ox t=-()z 10ρ ≈-'F H G I K J F H I K-z 1C t t Q t dx ox ox oxox oxSSt t t ∆∆b g =-'--=-'F H I K 1C Q t t t t Q C ox SS ox ox SSox ∆∆a for ∆V Q t FB SS ox ox=-'∈F H G I K J =-⨯⨯⨯⨯---()16108102001039885101910814...b g b g b gb gor∆V FB =-00742.V(b) We have ρx Q t SS ox()='=⨯⨯⨯--16108102001019108.b g b g =⨯=-64103.ρONow ∆V C x x t dx C t xdx FB oxox oxOox oxoxt t =-=-()zz10ρρor ∆V t FB O oxox=-∈ρ22=-⨯⨯⨯---()6410200102398851038214...bg b g b gor∆V FB =-00371.V (c) ρρx x t O ox()F H G I KJ =We find12216108102001019108t Q ox O SS O ρρ='⇒=⨯⨯⨯--.b gb g or ρO =⨯-128102. Now ∆V C t x x t dx FB ox ox O ox t ox =-⋅⋅F H G I KJ z110ρ =-⋅z122C t x dx ox O oxox t ρaf which becomes ∆V t t x t FB ox oxO oxox O oxox t =-∈⋅⋅=-∈F H G I KJ 1332302ρρaf Then∆V FB =-⨯⨯⨯---()12810200103398851028214...b g b g b gor 0494.0-=∆FB V V_______________________________________10.27 Sketch_______________________________________10.28 Sketch_______________________________________10.29 (b)⎪⎪⎭⎫⎝⎛-=-=2ln i d a t bi FB n N N V V V ()()()()⎥⎥⎦⎤⎢⎢⎣⎡⨯-=2101616105.11010ln 0259.0or695.0-=FB V V(c) Apply 3-=G V V, 3≅ox V VFor 3+=G V V,sdx d ∈-=Eρ n-side: d eN =ρ1C x eN eN dx d sd s d +∈-=E ⇒∈-=E0=E at n x x -=, then snd x eN C ∈-=1 so()n s dx x eN +∈-=E for 0≤≤-x x n In the oxide, 0=ρ, so=E ⇒=E 0dxd constant. From the boundary conditions, in the oxidesn d x eN ∈-=E In the p-region,2C x eN eN dx d sa sa s+∈=E ⇒∈+=∈-=Eρ 0=E at ()p ox x t x +=, then ()[]x x teN p oxsa-+∈-=EAt ox t x =, snd sp a x eN x eN ∈-=∈-=E So that n d p a x N x N = Since d a N N =, then p n x x = The potential is ⎰E -=dx φFor zero bias, we can write bi p ox n V V V V =++where p ox n V V V ,, are the voltage drops acrossthe n-region, the oxide, and the p-region, respectively. For the oxide:soxn d ox ox t x eN t V ∈=⋅E =For the n-region:()C x x x eN x V n s d n '+⎪⎪⎭⎫ ⎝⎛⋅+∈=22Arbitrarily, set 0=n V at n x x -=, thensnd x eN C ∈='22so that()()22n sdn x x eN x V +∈=At 0=x , snd n x eN V ∈=22which is the voltagedrop across the n-region. Because ofsymmetry, p n V V =. Then for zero bias, wehavebi ox n V V V =+2 which can be written as bi sox n d s n d V t x eN x eN =∈+∈2or 02=∈-+ds bi ox n n eN V t x x Solving for n x , we obtain dbis ox ox n eN V t t x ∈+⎪⎪⎭⎫ ⎝⎛+-=222 If we apply a voltage G V , then replace bi V by G bi V V +, so ()dG bi s ox ox p n eN V V t t x x +∈+⎪⎪⎭⎫ ⎝⎛+-==222 We find2105008-⨯-==p n x x()()()()()1619142810106.1695.31085.87.11210500---⨯⨯+⎪⎪⎭⎫ ⎝⎛⨯+ which yields510646.4-⨯==p n x x cmNow soxn d ox t x eN V ∈=()()()()()()148516191085.87.111050010646.410106.1----⨯⨯⨯⨯=or359.0=ox V V We also findsnd p n x eN V V ∈==22()()()()()142516191085.87.11210646.410106.1---⨯⨯⨯=or67.1==p n V V V_______________________________________10.30(a) n-type (b) We have731210110210200---⨯=⨯⨯=ox C F/cm 2Also ()()7141011085.89.3--⨯⨯=∈=⇒∈=ox ox ox ox ox ox C t t C or 61045.3-⨯=ox t cm 5.34=nm o A 345= (c)oxssms FB C Q V '-=φ or 71050.080.0-'--=-ssQwhich yields8103-⨯='ssQ C/cm 21110875.1⨯=cm 2- (d) ⎪⎪⎭⎫ ⎝⎛∈⎪⎭⎫ ⎝⎛⎪⎪⎭⎫ ⎝⎛∈∈+∈='d s s ox ox ox FB eN e kT t C()()[][6141045.31085.89.3--⨯÷⨯= ()()()()()⎥⎥⎦⎤⨯⨯⨯⎪⎭⎫ ⎝⎛+--161914102106.11085.87.110259.07.119.3 which yields81082.7-⨯='FBC F/cm 2 or156=FB C pF_______________________________________10.31 (a) Point 1: Inversion 2: Threshold3: Depletion4: Flat-band5: Accumulation_______________________________________10.32 We have ()()[]fp ms x GS ox nV V C Q φφ2+---=' ()()max SD ssQ Q '+'- Now let DS x V V =, so ()⎩⎨⎧--='DS GS ox n V V C Q ()()⎪⎭⎪⎬⎫⎥⎥⎦⎤⎢⎢⎣⎡+-'+'+fp ms ox ss SD C Q Q φφ2m ax For a p-type substrate, ()max SDQ ' is a negative value, so we can write()⎩⎨⎧--='DS GS ox n V V C Q()⎪⎭⎪⎬⎫⎥⎥⎦⎤⎢⎢⎣⎡++'-'-fp ms ox ss SD C Q Q φφ2m ax Using the definition of threshold voltage T V ,we have()[]T DS GS ox nV V V C Q ---=' At saturation()T GS DS DS V V sat V V -== which then makes nQ 'equal to zero at the drain terminal._______________________________________10.33(a) ()[]222DS DS T GS n D V V V V L W k I --⋅'= ()()()()[]22.02.04.08.028218.0--⎪⎭⎫ ⎝⎛= 0864.0=mA (b) ()22T GS n D V V LW k I -⋅'= ()()24.08.08218.0-⎪⎭⎫ ⎝⎛= 1152.0=mA(c) Same as (b), 1152.0=D I mA(d) ()22T GS n D V V L W k I -⋅'=()()24.02.18218.0-⎪⎭⎫ ⎝⎛= 4608.0=mA _______________________________________ 10.34 (a) ()[]222SDSD T SG p D V V V V LW k I -+⋅'= ()()()()[]225.025.04.08.0215210.0--⎪⎭⎫ ⎝⎛= 103.0=D I mA(b) ()22T SG p D V V LW k I +⋅'= ()()24.08.015210.0-⎪⎭⎫ ⎝⎛= 12.0=mA(c) ()22T SG p D V V L W k I +⋅'=()()24.02.115210.0-⎪⎭⎫ ⎝⎛=48.0=mA(d) Same as (c), 48.0=D I mA_______________________________________10.35(a) ()22T GS n D V V LW k I -⋅'=()28.04.126.00.1-⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛=L W26.9=⇒LW(b) ()()28.085.126.926.0-⎪⎭⎫ ⎝⎛=D I06.3=mA(c) ()[]222DSDS T GS n D V V V V L W k I --⋅'= ()()()()[]215.015.08.02.1226.926.0--⎪⎭⎫ ⎝⎛=271.0=mA_______________________________________10.36(a) Assume biased in saturation region()22T SG p D V V L W k I +⋅'=()()2020212.010.0T V +⎪⎭⎫ ⎝⎛=289.0+=⇒T V VNote: 0.1=SD V V 289.00+=+>T SG V V V So the transistor is biased in the saturation region.(b) ()()2289.04.020212.0+⎪⎭⎫ ⎝⎛=D I570.0=mA(c) ()()[()15.0289.06.0220212.0+⎪⎭⎫⎝⎛=D I()]215.0-or293.0=D I mA_______________________________________10.37 ()()781410138.3101101085.89.3---⨯=⨯⨯=ox C F/cm 2 ()()()()2.122010138.342527-⨯==L W C K ox n n μ310111.1-⨯=A/V 2=1.111 mA/V 2(a) 0=GS V , 0=D I 6.0=GS V V, ()15.0=sat V DS V, ()()()245.06.0111.1-=sat I D 025.0=mA2.1=GS V V, ()75.0=sat V DS V, ()()()245.02.1111.1-=sat I D 625.0=mA8.1=GS V V, ()35.1=sat V DS V,()()()245.08.1111.1-=sat I D 025.2=mA4.2=GS V V, ()95.1=sat V DS V,()()()245.04.2111.1-=sat I D 225.4=mA (c)0=D I for 45.0≤GS V V 6.0=GS V V,()()()()[]21.01.045.06.02111.1--=D I 0222.0=mA 2.1=GS V V,()()()()[]21.01.045.02.12111.1--=D I 156.0=mA 8.1=GS V V,()()()()[]21.01.045.08.12111.1--=D I 289.0=mA 4.2=GS V V,()()()()[]21.01.045.04.22111.1--=D I 422.0=mA_______________________________________10.38()()814101101085.89.3--⨯⨯=∈=ox ox ox t C 710138.3-⨯=F/cm 2L WC K ox p p 2μ=()()()()2.123510138.32107-⨯=41061.9-⨯=A/V 2=0.961 mA/V 2(a) 0=SG V , 0=D I6.0=SG V V, ()25.0=sat V SD V()()()235.06.0961.0-=sat I D 060.0=mA2.1=SG V V, ()85.0=sat V SD V()()()235.02.1961.0-=sat I D 694.0=mA 8.1=SG V V, ()45.1=sat V SD V()()()235.08.1961.0-=sat I D02.2=mA4.2=SG V V, ()05.2=sat V SD V()()()235.04.2961.0-=sat I D04.4=mA (c)0=D I for 35.0≤SG V V6.0=SG V V()()()()[]21.01.035.06.02961.0--=D I 0384.0=mA 2.1=SG V V ()()()()[]21.01.035.02.12961.0--=D I154.0=mA8.1=SG V V ()()()()[]21.01.035.08.12961.0--=D I 269.0=mA 4.2=SG V V()()()()[]21.01.035.04.22961.0--=D I 384.0=mA_______________________________________10.39(a) From Problem 10.37,111.1=n K mA/V 2 For 8.0-=GS V V, 0=D I0=GS V , ()8.0=sat V DS V()()()28.00111.1+=sat I D 711.0=mA8.0+=GS V V, ()6.1=sat V DS V()()()28.08.0111.1+=sat I D 84.2=mA6.1=GS V V, ()4.2=sat V DS V()()()28.06.1111.1+=sat I D 40.6=mA_______________________________________10.40 Sketch _______________________________________10.41 Sketch _______________________________________ 10.42We have ()T DS T GS DS V V V V sat V -=-=so that()T DS DS V sat V V +=Since ()sat V V DS DS >, the transistor is always biased in the saturation region. Then()2T GS n D V V K I -=where, from Problem 10.37,111.1=n K mA/V 2and 45.0=T V V10.43From Problem 10.38, 961.0=p K mA/V 2()()[]22SD SD T SG p D V V V V K I -+=()T SG p V SDDd V V K V I g SD +=∂∂=→20For 35.0≤SG V V, 0=d g For 35.0>SG V V,()()35.0961.02-=SG d V g For 4.2=SG V V,()()35.04.2961.02-=d g 94.3=mA/V_______________________________________10.44(a) GS D m V I g ∂∂=()()[]{}22DS DS T GS n GSV V V V K V --∂∂=()DS n V K 2=()()05.0225.1n K =5.12=⇒n K mA/V 2(b) ()()()[()]205.005.03.08.025.12--=D I 594.0=mA(c) ()()23.08.05.12-=D I125.3=mA_______________________________________10.45We find that 2.0≅T V V Now ()()T GS oxn D V V LC W sat I -⋅=2μ where ()()814104251085.89.3--⨯⨯=∈=ox ox oxt C or81012.8-⨯=ox C F/cm 2We are given 10=L W . From the graph, for 3=GS V V, we have ()033.0≅sat I D , then ()2.032033.0-⋅=LC W oxn μ or310139.02-⨯=LC W oxn μor()()3810139.01012.81021--⨯=⨯n μwhich yields342=n μcm 2/V-s_______________________________________10.46 (a)()T GS DS V V sat V -= or8.48.04=⇒-=GS GS V V V(b) ()()()sat V K V V K sat I DS n T GS n D 22=-= so()244102n K =⨯- which yields μ5.12=n K A/V 2 (c) ()2.18.02=-=-=T GS DS V V sat V Vso ()sat V V DS DS > ()()()258.021025.1-⨯=-sat I Dor ()μ18=sat I D A(d)()sat V V DS DS <()[]22DS DS T GS n D V V V V K I --= ()()()()[]25118.0321025.1--⨯=-orμ5.42=D I A_______________________________________10.47(a) ()()814101801085.89.3--⨯⨯=ox C 7109175.1-⨯=F/cm 2(i)()()7109175.1450-⨯=='ox n nC k μ 510629.8-⨯=A/V 2 or μ29.86='nk A/V 2 (ii)()()22T GS nD V V L W k sat I -⎪⎭⎫ ⎝⎛⎪⎪⎭⎫ ⎝⎛'= ()24.02208629.08.0-⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛=L W24.7=⇒L W(b) (i) ()()7109175.1210-⨯=='ox p p C k μ 510027.4-⨯=A/V 2or μ27.40='p k A/V 2(ii) ()()22T SG p D V V L W k sat I +⎪⎭⎫ ⎝⎛⎪⎪⎭⎫ ⎝⎛'= ()24.02204027.08.0-⎪⎭⎫ ⎝⎛⎪⎭⎫ ⎝⎛=L W5.15=⇒LW_______________________________________ 10.48 From Problem 10.37, 111.1=n K mA/V 2(a) ()()[]{}22DS DS T GS n GS mL V V V V K V g --∂∂= ()()()()1.02111.12==DS n V K so 222.0=mL g mA/V (b) (){}2T GS n GS ms V V K V g -∂∂=()()()45.05.1111.122-=-=T GS n V V K so 33.2=ms g mA/V _______________________________________10.49From Problem 10.38, 961.0=p K mA/V 2(a) ()()[]{}22SD SD T SG p SGmL V V V V K Vg -+∂∂= ()()()()1.02961.02==SD p V K or 192.0=mL g mA/V (b) ()[]2T SG p SGms V V K V g +∂∂=()()()35.05.1961.022-=+=T SG p V V K or 21.2=ms g mA/V_______________________________________10.50 (a) oxa s C N e ∈=2γNow ()()814101501085.89.3--⨯⨯=oxC 710301.2-⨯=F/cm 2 Then()()()()716141910301.21051085.87.11106.12---⨯⨯⨯⨯=γ 5594.0=γV 2/1 (b) ()3890.0105.1105ln 0259.01016=⎪⎪⎭⎫⎝⎛⨯⨯=fpφV (i)()()()()()2/1161914105106.13890.01085.87.114⎥⎦⎤⎢⎣⎡⨯⨯⨯=--dT x510419.1-⨯=cm()m ax SDQ ' ()()()5161910419.1105106.1--⨯⨯⨯=710135.1-⨯=C/cm 2 ()fp FB oxSDTO V C Q V φ2max ++'= ()3890.025.010301.210135.177+-⨯⨯=-- 7713.0=VL WC K ox n n 2μ=()()()()2.12810301.24507-⨯=410452.3-⨯=A/V 2 or 3452.0=n K mA/V 2 For 0=D I , 7713.0==TO GS V V V For 5.0=D I ()()27713.03452.0-=GS V 975.1=⇒GS V V (c) (i) For 0=SB V , 7713.0==TO T V V V (ii) 1=SB V V,()()[1389.025594.0+=∆T V()]389.02-2525.0=V024.12525.07713.0=+=T V V (iii) 2=SB V V,()()[2389.025594.0+=∆T V ()]389.02-4390.0=V210.14390.07713.0=+=T V V (iv) 4=SB V V,()()[4389.025594.0+=∆T V()]389.02-7294.0=V501.17294.07713.0=+=T V V _______________________________________10.51()3473.0105.110ln 0259.01016=⎪⎪⎭⎫⎝⎛⨯=fp φ V[]fpSBfpT V V φφγ22-+=∆()()[5.23473.0212.0+=()]3473.02- or114.0=∆T V VNow T TO T V V V ∆+= 114.05.0+=TO V 386.0=⇒TO V V _______________________________________ 10.52 (a) ()()814102001085.89.3--⨯⨯=ox C710726.1-⨯=F/cm 2oxds C N e ∈=2γ ()()()()715141910726.11051085.87.11106.12---⨯⨯⨯⨯= 2358.0=γV 2/1 (b) ()3294.0105.1105ln 0259.01015=⎪⎪⎭⎫⎝⎛⨯⨯=fnφV []fn BS fnT V V φφγ22-+-=∆()()[BS V +-=-3294.022358.022.0()]3294.02- 39.2=⇒BS V V_______________________________________10.53(a) +n poly-to-p-type 0.1-=⇒ms φV ()288.0105.110ln 0259.01015=⎪⎪⎭⎫⎝⎛⨯=fp φValso 2/14⎥⎦⎤⎢⎣⎡∈=a fp s dTeN x φ()()()()()2/115191410106.1288.01085.87.114⎥⎦⎤⎢⎣⎡⨯⨯=-- or410863.0-⨯=dT x cm Now()()()()4151910863.010106.1m ax --⨯⨯='SDQ or()81038.1m ax -⨯='SDQ C/cm 2 Also()()814104001085.89.3--⨯⨯=∈=ox ox ox t C or81063.8-⨯=ox C F/cm 2 We find ()()91019108105106.1--⨯=⨯⨯='ss Q C/cm 2 Then ()fp ms oxss SD T C Q Q V φφ2m ax ++'-'=()288.020.11063.81081038.1898+-⎪⎪⎭⎫ ⎝⎛⨯⨯-⨯=--- or 357.0-=T V V(b) For NMOS, apply SB V and T V shifts in apositive direction, so for 0=T V , we want 357.0+=∆T V V. So[]fp SB fpoxa s T V C N e V φφ222-+∈=∆or()()()()81514191063.8101085.87.11106.12357.0---⨯⨯⨯=+ ()()[]288.02288.02-+⨯SB V or[]576.0576.0211.0357.0-+=SB V which yields 43.5=SB V V_______________________________________10.54 Plot_______________________________________10.55 (a)()T GS oxn m V V L C W g -=μ()T GS oxoxn V V t L W -∈=μ ()()()()()65.0510*******.89.340010814-⨯⨯=--or26.1=m g mS Nowsm m m s m m m r g g g r g g g +=='⇒+='118.01which yields⎪⎭⎫⎝⎛-=⎪⎭⎫ ⎝⎛-=18.0126.1118.011m s g r or 198.0=s r k Ω (b) For 3=GS V V, 683.0=m g mS Then ()()602.0198.0683.01683.0=+='m g mS or 88.0683.0602.0=='m m g g which is a 12% reduction._______________________________________10.56 (a) The ideal cutoff frequency for no overlap capacitance is,()222L V V C g f T GS n gs m T πμπ-==()()()24102275.04400-⨯-=π or 17.5=T f GHz (b) Now ()M gsT m T C C g f +=π2 where ()L m gdT M R g C C +=1 We find()()4410201075.0--⨯⨯=ox gdT C C()()814105001085.89.3--⨯⨯= ()()4410201075.0--⨯⨯⨯ or1410035.1-⨯=gdT C F Also ()T GS oxn m V V LC W g -=μ()()()()()()84144105001021085.89.34001020----⨯⨯⨯⨯= ()75.04-⨯or3108974.0-⨯=m g SThen ()1410035.1-⨯=M C ()()[]331010108974.01⨯⨯+⨯- or 1310032.1-⨯=M C F Now()()W L C C ox gsT 41075.0-⨯+= ()()814105001085.89.3--⨯⨯= ()()44410201075.0102---⨯⨯+⨯⨯ or1410797.3-⨯=gsT C F We now find ()M gsT mTC C g f +=π2 ()1314310032.110797.32108974.0---⨯+⨯⨯=π or 01.1=T f GHz _______________________________________10.57 (a) For the ideal case()4610221042-⨯⨯==ππυL f ds Tor 18.3=T f GHz(b) With overlap capacitance (using the values from Problem 10.56), ()MgdT mT C C g f +=π2 We findds ox m W C g υ= ()()()()86144105001041085.89.31020---⨯⨯⨯⨯= or3105522.0-⨯=m g S We have()L m gdT M R g C C +=1 ()1410035.1-⨯=()()[]331010105522.01⨯⨯+⨯- or 1410750.6-⨯=M C F。

半导体物理与器件第四版课后习题答案

半导体物理与器件第四版课后习题答案第一章半导体材料基础知识1.1 小题一根据题目描述，当n=5时，半导体材料的载流子浓度为’n=2.5×1015cm(-3)’，求势垒能为多少？解答：根据势垒能公式E_g = E_c - E_v其中E_g为势垒能，E_c为导带底，E_v为价带顶。

根据载流子浓度和温度的关系n = 2 * （2 * pi * m_e * k * T / h^2）^(3/2) * e^(-E_g / (2 * k * T))其中m_e为载流子质量，k为玻尔兹曼常数，T为绝对温度。

可以得到E_g = -2 * k * T * ln(n / (2 * （2 * pi * m_e * k * T / h^2）^(3/2)))代入已知条件，计算得到势垒能为E_g = -2 * 1.38 * 10^(-23) * 300 * ln(2.5 * 10^15 / (2 * （2 * pi * 9.1 * 10^(-31) * 1.38 * 10^ (-23) * 300 / (6.63 * 10^(-34))^2）^(3/2)))1.1 小题二根据题目描述，当势垒能E_g=1.21eV时，求温度为多少时，载流子浓度为’n=5.0×1015cm(-3)’？解答：按照1.1 小题一的公式，可以求出温度TT = E_g / (2 * k * ln(n / (2 * （2 * pi * m_e * k * T / h^2）^(3/2))))将已知数据代入公式，计算得到温度T = 1.21 / (2 * 1.38 * 10^(-23) * ln(5 * 10^15/ (2 * （2 * pi * 9.1 * 10^(-31) * 1.38 * 10^(-2 3) * T / (6.63 * 10^(-34))^2）^(3/2))))第二章半导体材料与器件基本特性2.1 小题一根据题目描述，当Si掺杂浓度[N_b]为5×10^15 cm(-3)和[P_e]为2×1017 cm^(-3)，求Si中的载流子浓度和导电类型。

半导体物理与器件第四版课后习题答案8

Chapter 8In forward biasThenor(a)For , thenormVmV(b)For , thenormVmV_______________________________________ 8.2cmcm(a) V,cmorcm(b) V,cmcm(c) V_______________________________________ 8.3cmcm(a) V,cmcm(b) Vcmcm(c)_______________________________________8.4(a)cmcm(i)orV(ii) n-region - lower doped side(b)cmcm(i)V(ii) p-region - lower doped side_______________________________________8.5(a)A/cmAor mA(b)A/cmAor mA(c) mA_______________________________________ 8.6For an silicon diodeorA(a) For V,A(b) For V,orA_______________________________________8.7A/cm(a)Aor mA(b)A_______________________________________8.8(a)A/cmA(i)A(ii)A(iii)A_______________________________________ 8.9We haveor we can write this asso thatIn reverse bias, is negative, so at, we haveormV_______________________________________ 8.10Case 1:AmAmA/cmCase 2:or mAmA/cmCase 3:SoVThenmACase 4:mAcm_______________________________________8.11(a)or(b) From part (a),or_______________________________________ 8.12The cross-sectional area iscmWe havewhich yieldsA/cmWe can writeWe wantor=which yieldsNowWe findcmandcm_______________________________________ 8.13Plot_______________________________________8.14(a)We haveandsoor(b) Using Einstein's relation, we can writeWe haveandAlsoThen_______________________________________8.15(a) p-side;oreVAlso on the n-side;oreV(b) We can findcm/scm/sNoworA/cmThenorAWe findorAA(c) The hole current isor(A)Then_______________________________________8.16(a)A(b)A(c)VVcm(d)A(e)AANowAThenA_______________________________________8.17(a) The excess hole concentration is given by We findcmandcm mThenorcm(b) We haveAt cm,orA/cm(c) We haveWe can determine thatcm and mThenorA/cmWe can also findA/cmThen at m,orA/cm_______________________________________ 8.188. (a) Problem 8.7orV(b) Problem 8.8orV_______________________________________ 8.19The excess electron concentration is given by The total number of excess electrons isWe may note thatThenWe find thatcm/s and mAlsocmThenorThen, we find the total number of excesselectrons in the p-region to be:(a)V,(b)V,(c)V,Similarly, the total number of excess holes in the n-region is found to beWe find thatcm/s and mAlsocmThenSo(a)V,(b)V,(c)V,_______________________________________ 8.20ThensoorWe then haveorThenoreV_______________________________________ 8.21(a) We havewhich can be written in the formor(b) Taking the ratioFor K, ,For K, ,(i) Germanium: eVor(ii) Silicon: eVor_______________________________________ 8.22Plot_______________________________________ 8.23First case:or VNowKSecond case:orNowBy trial and error,KThe reverse-bias current is limiting factor._______________________________________ 8.24cmor m;(a)(i)orV(ii)AAAor mA(b) (i)orV(ii)AAAor mA_______________________________________8.25(a) We can write for the n-regionThe general solution is of the formThe boundary condition at givesand the boundary condition atgivesFrom this equation, we haveThen, from the first boundary condition, weobtainWe then obtainwhich can be written asWe can also findThe solution can now be written asor finally(b)=Then_______________________________________ 8.26For the temperature range K,neglect the change in and .ThenTaking the ratio of currents, but maintaining a constant, we haveWe then haveWe haveK , V andeV, VK ,eV, VK ,eV, VFor K ,which yieldsVFor K ,which yieldsV_______________________________________8.27(a) We can writewhere C is a constant, independent oftemperature.As a first approximation, neglect thevariation of and with temperatureover the range of interest. We can then write where is another constant, independent oftemperature. We findor_______________________________________8.28(a)A(b)We findVandcmThenA(c)_______________________________________8.29(a) Set ,socmThenBy trial and error,KWe haveThenAor A(b) From Problem 8.28AASoV_______________________________________ 8.30cm/scm/s(a)(i)A(ii)A(iii)A(iv)A(b)Vcm(i)ThenA(ii)A(iii)A(iv)A_______________________________________ 8.31Using results from Problem 8.30, we findV, A,A, AV, AA, AV, AA, AV, AA, AV. AA, A_______________________________________ 8.32Plot_______________________________________ 8.33Plot_______________________________________ 8.34We have thatLet andWe can writeandWe also haveso thatThenDefineandThen the recombination rate can be written as orTo find the maximum recombination rate, set orwhich simplifies toThe denominator is not zero, so we haveorThen the maximum recombination ratebecomesorwhich can be written asIf , then we can neglect the (-1)term in the numerator and the (+1) term in the denominator, so we finally haveQ.E.D. _______________________________________ 8.35We haveIn this case, cms and isa constant through the space charge region. ThenorVAlsoorcmThenorA/cm_______________________________________8.36orA/cmNoworwhich can be written asWe findorV_______________________________________8.37(a)For nF(b)For nF_______________________________________8.38(a) , For mAC(b) For mAC_______________________________________8.39For a diode,NowSandFWe havewhereWe obtainkHz ,kHz ,MHz ,MHz ,_______________________________________ 8.40Reverse biasVF(V) (pF)10 1.5555 2.1233 2.6241 3.8180 5.7476.6508.179Forward biasForThenA(V) (F) + (F) = (F)0.20.40.60_______________________________________ 8.41For a diode, , thenNowF/AThenorsAt 1 mA,orF_______________________________________8.42(a)(i)orAor mA(ii)V(iii)(b)(i)Aor mA(ii)V(iii)_______________________________________8.43(a) p-region:soorn-region:soorThe total resistance isor(b)which yieldsmA_______________________________________ 8.44orWe can write(a) (i) For mA,or V(ii) For mA,or V(b) Set(i) For mA,or V(ii) For mA,or V_______________________________________8.45(a)or AV(b) AV_______________________________________8.46(a)orwhich yields(b)which yields_______________________________________8.47(a) IfThen we haveorWe find(b) If , thenwhich yields_______________________________________8.48(a) erferf= erferfThen(b) erfBy trial and error,_______________________________________ 8.49pF atpF at VWe haves , mAandmASoorsAlsopFThe time constant issNow, the turn-off time iss_______________________________________ 8.50VWe findwhich yieldscm_______________________________________ 8.51Sketch_______________________________________ 8.53From Figure 7.15, cmLet cmcmThen VAor cm_______________________________________。

半导体物理与器件第四版课后习题答案（供参考）

半导体物理与器件第四版课后习题答案（供参考）Chapter 44.1 where cO N and O N υ are the values at 300 K.4.2Plot_______________________________________4.3By trial and error, 5.367?T K(b)By trial and error, 5.417?T K_______________________________________4.4At 200=T K, ()=3002000259.0kT017267.0=eVAt 400=T K, ()?=3004000259.0kT 034533.0=eVoror 318.1=g E eV Now so 371041.9?=o co N N υcm 6-_______________________________________4.5 For 200=T K, 017267.0=kT eV For 300=T K, 0259.0=kT eVFor 400=T K, 034533.0=kT eV(a) For 200=T K,(b) For 300=T K,(c) For 400=T K,_______________________________________4.6Let x E E c =-Then ??-∝kT x x f g F c exp To find the maximum value: which yields The maximum value occurs at (b)Let x E E =-υ Then ()??-∝-kT x x f g F exp 1υ To find the maximum value Same as part (a). Maximum occurs at or_______________________________________4.7 wherekT E E c 41+= and 22kT E E c += Then or _______________________________________ 4.8 Plot_______________________________________ 4.9 Plot _______________________________________4.10 Silicon: o p m m 56.0*=, o n m m 08.1*=0128.0-=-midgap Fi E E eV Germanium: o p m m 37.0*=,o n m m 55.0*=0077.0-=-midgap Fi EE eVGallium Arsenide: o p m m 48.0*=, 0382.0+=-midgapFi E E eV _______________________________________4.12 63.10-?meV 47.43+?meV _______________________________________ 4.13 Let ()==K E g c constant Then Let kTE E c-=η so that ηd kT dE ?= We can writeso that The integral can then be written as which becomes_______________________________________ 4.14Let ()()c c E E C E g -=1 for c E E ≥ Then LetkTE E c-=η so that ηd kT dE ?=We can write Then orWe find that So_______________________________________ 4.15We have=∈*1m m a r o r o For germanium, 16=∈r , o m m 55.0*= Then orThe ionization energy can be written as ()6.132*∈∈???? ??=s o o m m E eV ()()029.06.131655.02=?=E eV_______________________________________ 4.16We have=∈*1m m a r o r o For gallium arsenide, 1.13=∈r , Then The ionization energy is or0053.0=E eV_______________________________________ 4.17 2148.0=eV90518.02148.012.1=-=eV 31090.6?=cm 3- (a) Holes 338.0=eV_______________________________________ 4.18 162.0=eV 958.0162.012.1=-=eV 31041.2?=cm 3-365.0=eV_______________________________________ 4.19 8436.0=eV2764.0=-υE E F eV1410414.2?=cm 3- (a) p-type_______________________________________ 4.20 (a) ()032375.03003750259.0==kT eV141015.1?=cm 3-14.1=eV31099.4?=cm 3-2154.0=eV 2046.1=eV 21042.4-?=cm 3-_______________________________________ 4.21 (a) ()032375.03003750259.0==kT eV151086.6?= cm 3-840.0=eV 71084.7?=cm 3-2153.0=eV9047.02153.012.1=-=-υE E F eV 31004.7?=cm 3-_______________________________________ 4.22(a) p-type(b) 28.0412.14===-g F E E E υeV141010.2?=cm 3- 84.028.012.1=-=eV 51030.2?=cm 3-_______________________________________ 4.23 131033.7?=cm 3- 61007.3?=cm 3- 91080.8?=cm 3-21068.3?=cm 3-_______________________________________ 4.241979.0=eV 92212.019788.012.1=-=eV 31066.9?=cm 3- (a) Holes 3294.0=eV _______________________________________。

1、下载文档前请自行甄别文档内容的完整性，平台不提供额外的编辑、内容补充、找答案等附加服务。
2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
3、如文档侵犯您的权益，请联系客服反馈,我们会尽快为您处理(人工客服工作时间：9:00-18:30)。

半导体物理与器件第四版答案【篇一：半导体物理第五章习题答案】>1. 一个n型半导体样品的额外空穴密度为1013cm-3，已知空穴寿命为100?s，计算空穴的复合率。

解：复合率为单位时间单位体积内因复合而消失的电子-空穴对数，因此1013u1017cm?3?s ?6100102. 用强光照射n型样品，假定光被均匀吸收，产生额外载流子，产生率为gp，空穴寿命为?，请①写出光照开始阶段额外载流子密度随时间变化所满足的方程；②求出光照下达到稳定状态时的额外载流子密度。

解：⑴光照下，额外载流子密度?n=?p，其值在光照的开始阶段随时间的变化决定于产生和复合两种过程，因此，额外载流子密度随时间变化所满足的方程由产生率gp和复合率u的代数和构成，即 d(?p)?pgp dtd(?p)0，于是由上式得⑵稳定时额外载流子密度不再随时间变化，即 dtppp0gp3. 有一块n型硅样品，额外载流子寿命是1?s，无光照时的电阻率是10??cm。

今用光照射该样品，光被半导体均匀吸收，电子-空穴对的产生率是1022/cm3?s，试计算光照下样品的电阻率，并求电导中少数载流子的贡献占多大比例？解：光照被均匀吸收后产生的稳定额外载流子密度pngp10221061016 cm－3取?n?1350cm2/(v?s)，?p?500cm/(v?s)，则额外载流子对电导率的贡献2pq(?n??p)?1016?1.6?10?19?(1350?500)?2.96 s/cm无光照时?0?10.1s/cm，因而光照下的电导率0?2.96?0.1?3.06s/cm相应的电阻率 ??110.33cm 3.06少数载流子对电导的贡献为：?p?pq?p??pq?p?gp?q?p代入数据：?p?(p0??p)q?p??pq?p?1016?1.6?10?19?500?0.8s/cm∴p00.80.2626﹪ 3.06即光电导中少数载流子的贡献为26﹪4.一块半导体样品的额外载流子寿命? =10?s，今用光照在其中产生非平衡载流子，问光照突然停止后的20?s时刻其额外载流子密度衰减到原来的百分之几？解：已知光照停止后额外载流子密度的衰减规律为p(t)p0e因此光照停止后任意时刻额外载流子密度与光照停止时的初始密度之比即为t??p(t)e p0t当t?20?s?2?10?5s时20??p(20)e10e20.13513.5﹪ ?p05. 光照在掺杂浓度为1016cm-3的n型硅中产生的额外载流子密度为?n=?p= 1016cm-3。

计算无光照和有光照时的电导率。

解：根据新版教材图4-14（a）查得nd=1016cm-3的n型硅中多子迁移率n1100cm2/(vs)少子迁移率p500cm2/(vs)设施主杂质全部电离，则无光照时的电导率0n0qn10161.6101911001.76 s/cm有光照时的电导率0??nq(?n??p)?1.76?1014?1.6?10?19?(1100?400)?1.784s/cm6.画出p型半导体在光照（小注入）前后的能带图，标出原来的费米能级和光照时的准费米能级。

ecefnef ev光照前能带图光照后（小注入）能带图注意细节：① p型半导体的费米能级靠近价带；②因为是小注入，?pp0，即p=(p0+?p)≈p0，因此efp非常靠近ef，但efp必须在ef之下，因为p 毕竟大于p0③即便是小注入，p型半导体中也必是?nn0，故efn要远比ef更接近导带，但因为是小注入，?n p0，所以efn距导带底的距离必大于ef距价带顶的距离。

上述带色字所强调的两个细节学生容易忽略，要多加关注。

efp7. 光照在施主浓度nd=1015cm-3的n型硅中产生额外载流子?n=?p=1014cm-3。

试计算这种情况下准费米能级的位置，并和原来的费米能级作比较。

解：设杂质全部电离，则无光照时n0?nd 由n0?nieeiefkt得光照前n01015ef?ei?ktln?ei?0.026ln?ei?0.289ev 10ni1.5?10光照后n?n0??n?1.1?1015cm?3,这种情况下的电子准费米能级n1.1?1015efn?ei?ktln?ei?0.026ln?ei?0.291 evni1.5?1010空穴准费米能级efpp1014ni1.5?10与ef相比，电子准费米能级之差efn?ef?0.002 ev，相差甚微；而空穴准费米能级之差ef?efp?0.518 ev，即空穴准费米能级比平衡费米能级下降了0.52ev。

由此可见，对n型半导体，小注入条件下电子准费米能级相对于热平衡费米能级的变化很小，但空穴准费米能级变化很大。

8. 在一块p型半导体中，有一种复合－产生中心，小注入时，被这些中心俘获的电子发射回导带的过程和它与空穴复合的过程具有相同的几率。

试求这种复合－产生中心的能级位置，并说明它能否成为有效的复合中心？解：用et表示该中心的能级位置，参照参考书的讨论，知单位时间单位体积中由et能级发射回导带的电子数应等于et上俘获的电子数nt与电子的发射几率s－之积（s－＝rnn1），与价带空穴相复合的电子数则为rppnt；式中，rpp可视为et能级上的电子与价带空穴相复合的几率。

由题设条件知二者相等，即rnn1?rpp式中n1?nceecetkt。

对于一般复合中心，rn?rp或相差甚小，因而可认为 n1＝p；再由小注入条件p=(p0+?p)≈p0，即得n1?p0即ec?etktef?evktnce由此知nvencnvet?ec?ev?ef?ktlnn1(ec?ev?k0tlnc) 2nv∵本征费米能级ei?∴上式可写成et?2ei?ef，或写成et?ei?ei?ef室温下， p型半导体ef一般远在ei之下，所以et远在ei之上，故不是有效复合中心。

10.一块n型硅内掺有1016cm-3的金原子，试求它在小注入时的寿命。

若一块p型硅内也掺有1016cm-3的金原子，它在小注入时的寿命又是多少？－解：n型si中金能级作为受主能级而带负电成为au，其空穴俘获率 rp?1.15?10?7cm3/s因而n型si中的少子寿命p11?108.7?10s ?716rpnt1.15?10?10＋p型si中金能级作为施主能级而带正电成为au，其电子俘获率rn?6.3?10?8cm3/s因而p型si中的少子寿命n111.59?10?9s ?816rnnt6.3?10?1011．在下述条件下，是否有载流子的净复合或者净产生：①载流子完全耗尽（即n，p都大大小于ni）的半导体区域。

②在只有少数载流子被耗尽（例如pnpn0而nn=nn0）的半导体区域。

③在n=p的半导体区域，这里nni。

解：⑴载流子完全耗尽即意味着n?ni，p?ni，np?ni2，因而额外载流子的复合率u?np?ni2p(nnieei?eck0t)??n(p?nieei?etk0t0 )即该区域产生大于复合，故有载流子净产生。

⑵若nn?nn0，pn?pn0，则nnpn?nn0pn0?ni，即np?ni2按上列复合率公式知该区域复合率u0，故有载流子净产生。

⑶若n?p且n?ni，则必有np?ni2，按上列复合率公式知该区域u0，即该区域有载流子的净复合。

212、对掺杂浓度nd =1016cm-3、少数载流子寿命?p=10?s的n型硅，求少数载流子全部被外界清除时电子-空穴对的产生率。

（设et=ei）解：在少数载流子全部被清除（耗尽）、即n型硅中p=0的情况下，通过单一复合中心进行的复合过程的复合率公式 (5-42) 变成ni2u?p(nni)nni式中已按题设et=ei代入了n1=p1=ni。

由于n=nd =1016cm-3，而室温硅的ni只有1010cm-3量级，因而n+nini，上式分母中的第二项可略去，于是得ni2-(1.51010)2931u2.25?10 cm?s?61610p(nni)1010(101.510)复合率为负值表示此时产生大于复合，电子-空穴对的产生率g??u?2.25?109 cm?3?s?1另解：若非平衡态是载流子被耗尽，则恢复平衡态的驰豫过程将由载流子的复合变为热激发产生，产生率与少子寿命的乘积应等于热平衡状态下的少数载流子密度，因此得(1.5?1010)2ni21ni22.25?109 cm?3?s?1 g?616ppn0pnd101010p0【篇二：半导体物理与器件习题】>1．如图是金刚石结构晶胞，若 a 是其晶格常数，则其原子密度是。

答：为了改变导电性而向半导体材料中加入杂质的技术称为掺杂。

常用的掺杂方法有扩散和离子注入。

6．什么是替位杂质？什么是填隙杂质？ 7．什么是晶格？什么是原胞、晶胞？第二章量子力学初步1．量子力学的三个基本原理是三个基本原理能量量子化原理、波粒二相性原理、不确定原理。

2．什么是概率密度函数？3．描述原子中的电子的四个量子数是：、、、。

第三章固体量子理论初步1．能带的基本概念能带（energy band）包括允带和禁带。

允带（allowed band）：允许电子能量存在的能量范围。

禁带（forbidden band）：不允许电子存在的能量范围。

允带又分为空带、满带、导带、价带。

空带（empty band）：不被电子占据的允带。

满带（filled band）：允带中的能量状态（能级）均被电子占据。

导带：有电子能够参与导电的能带，但半导体材料价电子形成的高能级能带通常称为导带。

价带:由价电子形成的能带，但半导体材料价电子形成的低能级能带通常称为价带。

2．什么是漂移电流？漂移电流：漂移是指电子在电场的作用下的定向运动，电子的定向运动所产生的电流。

3．什么是电子的有效质量？晶格中运动的电子，在外力和内力作用下有：Ｆ总＝Ｆ外＋Ｆ内=ma,m是粒子静止的质量。

Ｆ外=m*na, m*n称为电子的有效质量。

4．位于能带底的电子，其有效质量为正，位于能带顶电子，其有效质量为负。

5．在室温t=300k，si的禁带宽度：eg=1.12ev ge的禁带宽度：eg=0.67ev gaas的禁带宽度：eg=1.43eveg具有负温度系数，即t越大，eg越小；eg反应了，在相同温度下，eg越大，电子跃迁到导带的能力越弱。

6．在热平衡状态下，晶体中的电子在不同能量的量子态上统计分布几率是一定的，电子遵循费米统计律，电子的费米分布函数是：f(e)?1?exp(1e?efkt)0费米能级ef和温度、半导体材料的导电类型、杂质的含量有关。

处于热平衡状态的系统有统一的费米能级。

因此，在温度不很高时，能量大于费米能级的量子态基本没有被电子占据，而能量小于费米能级的量子态基本上为电子所占据；而电子占据费米能级的几率在各种温度下总是１/2.费米能级标志了电子填充能级的水平，比ef高的量子态，基本为空，而比ef底的量子态基本上全被电子所占满.这样费米能级ef就成为量子态是否被电子占据的分界线：1) 能量高于费米能级的量子态基本是空的； 2) 能量低于费米能级的量子态基本是满的；3) 能量等于费米能级的量子态被电子占据的几率是50%。