2014-2012加拿大滑铁卢大学11年级数学竞赛试题

合集下载

国际数学竞赛试题及答案

国际数学竞赛试题及答案一、选择题（每题3分，共15分）1. 下列哪个数是最小的正整数？A. 0B. 1C. 2D. 3答案：B2. 一个圆的半径为5，它的面积是多少？A. 25πB. 50πC. 100πD. 25答案：B3. 如果一个数的平方等于它本身，这个数可能是：A. 1B. -1C. 2D. 3答案：A B4. 一个数列的前三项是1, 1, 2，如果这个数列是等差数列，那么第四项是：A. 3B. 4C. 5D. 6答案：A5. 以下哪个表达式是正确的？A. sin²θ + cos²θ = 1B. sinθ + cosθ = 1C. sinθ * cosθ = 1D. tanθ = sinθ / cosθ答案：A D二、填空题（每题4分，共20分）6. 如果一个三角形的两边长分别是3和4，且这两边夹角为60度，那么第三边的长度是________。

答案：√137. 一个函数f(x) = 2x³ - 3x² + x - 5，求导后得到的导函数是________。

答案：6x² - 6x + 18. 一个数的立方根等于它本身，这个数可以是________。

答案：1, -1, 09. 一个正六边形的内角和是________。

答案：720°10. 如果一个等差数列的首项是2，公差是3，那么第10项是________。

答案：37三、解答题（每题10分，共30分）11. 证明：对于任意实数x，等式e^x ≥ x + 1成立。

证明：考虑函数f(x) = e^x - (x + 1)。

对f(x)求导得到f'(x) = e^x - 1。

当x > 0时，f'(x) > 0，说明f(x)在此区间单调递增；当x < 0时，f'(x) < 0，说明f(x)在此区间单调递减。

因此，f(x)的最小值出现在x = 0处，此时f(0) = e^0 - (0 + 1) = 1 - 1 = 0。

2013滑铁卢竞赛试题答案

The CENTRE for EDUCATION in MATHEMATICS and COMPUTING cemc.uwaterloo.ca
2013 Euclid Contest
Wednesday, April 17, 2013
(in North America and South America)
since AC > 0. Finally, the perimeter of
3. (a) The parity of an integer is whether it is even or odd. Since the Fibonacci sequence begins 1, 1, 2, 3, 5, 8, 13, 21, . . ., then the parities of the ﬁrst eight terms are Odd, Odd, Even, Odd, Odd, Even, Odd, Odd. In the sequence, if x and y are consecutive terms, then the next term is x + y . In general, suppose that x and y are integers. If x is even and y is even, then x + y is even. If x is even and y is odd, then x + y is odd. If x is odd and y is even, then x + y is odd. If x is odd and y is odd, then x + y is even. Therefore, the parities of two consecutive terms x and y in the Fibonacci sequence determine the parity of the following term x + y . Also, once there are two consecutive terms whose parities match the parities of two earlier consecutive terms in the sequence, then the parities will repeat in a cycle. In particular, the parities of the fourth and ﬁfth terms (Odd, Odd) are the same as the parities of the ﬁrst and second terms (Odd, Odd). Therefore, the parities in the sequence repeat the cycle Odd, Odd, Even. This cycle has length 3. Therefore, the 99th term in the Fibonacci sequence ends one of these cycles, since 99 is a multiple of 3. In particular, the 99th term ends the 33rd cycle. Each cycle contains two odd terms. Therefore, the ﬁrst 99 terms in the sequence include 2 × 33 = 66 odd terms. Finally, the 100th term in the sequence begins a new cycle, so is odd. Therefore, the ﬁrst 100 terms include 66 + 1 = 67 odd terms. (b) Suppose that the ﬁrst term in the given sequence is a and the common diﬀerence is d. Then the ﬁrst four terms are a, a + d, a + 2d, a + 3d. From the given information, a + (a + 2d) = 6 and (a + d) + (a + 3d) = 20. The ﬁrst equation simpliﬁes to 2a + 2d = 6 or a + d = 3. The second equation simpliﬁes to 2a + 4d = 20 or a + 2d = 10. Therefore, (a + 2d) − (a + d) = 10 − 3 or d = 7. Since a + d = 3 and d = 7, then a = −4. Therefore, the tenth term in the sequence is a + 9d = −4 + 9(7) = 59. 4. (a) There are ﬁve odd digits: 1, 3, 5, 7, 9. We consider the positive integers less than 1000 in three sets: those with one digit, those with two digits, and those with three digits. There are 5 positive one-digit integers with one odd digit (namely 1, 3, 5, 7, 9). Consider the two-digit positive integers with only odd digits. Such an integer has the form XY where X and Y are digits. There are ﬁve possibilities for each of X and Y (since each must be odd). Therefore, there are 5 × 5 = 25 two-digit positive integers with only odd digits. Consider the three-digit positive integers with only odd digits. Such an integer has the form XY Z where X , Y and Z are digits.

滑铁卢大学欧几里得数学竞赛

该考试是学生申请滑铁卢大学数学学院本科专业的重要参考。

众所周知滑铁卢大学数学学院是全球最大的数学、统计学、计算机科学等学科教学中心比尔•盖茨曾于 2005 年、 2008 年两度造访该大学是比尔•盖茨大学巡回讲座的北美5 所大学之一也是唯一的一所加拿大大学。

考试范围：大部分的题目基于高三或者12年级数学课学习的内容。

我们的竞赛题目主要包括以下的数学内容：Ø 欧几里德几何和解析几何Ø 三角函数，包括函数、图像、性质、正弦余弦定理Ø 指数和对数函数Ø 函数符号Ø 方程组Ø 多项式，包括二次三次方程根的关系、余数定理Ø 数列、数列求和Ø 简单的计算问题Ø 数字的性质考试时间为 2.5 个小时， 10 道题。

每题 10 分，共计 100 分。

考试题有两种，一种只需要给出答案，另一种则需要写出整个解题过程，这种题的最终得分不仅取决于结果正确与否，还与解题思路有关。

Ø 笔试Ø 10道题：大部分要求写出完整的解题步骤；Ø 根据解题的方法和步骤获得相应的分数；Ø 步骤不完整的解题无法得到全部的分数；Ø 竞赛时长为2.5小时；Ø 共100分；Ø 可以使用无编程无绘图功能的计算器；Ø 不可以使用任何可接入互联网的设备，如手机、平板电脑等均不能携带如何准备：Ø CEMC官网可以免费下载历年的竞赛原题以及标准答案；Ø CEMC官网提供各种免费的数学资源；Ø www.cemc.uwaterloo.ca；如何参加：Ø 学校可以申请注册为考点，安排组织欧几里德数学竞赛；Ø 学生需要通过自己所在的学校报名参加欧几里德数学竞赛；Ø 如果学生所在学校未注册考点，学生可以报名在我们北京或者上海的考点参加欧几里德数学竞赛；Ø 竞赛结束之后，学校需要将全部的试卷寄回滑铁卢大学；Ø 改卷结束之后，滑铁卢大学会在CEMC官网录入学生的成绩。

Fryer滑铁卢数学竞赛(Grade 9)-数学Mathematics-2010-试题 exam

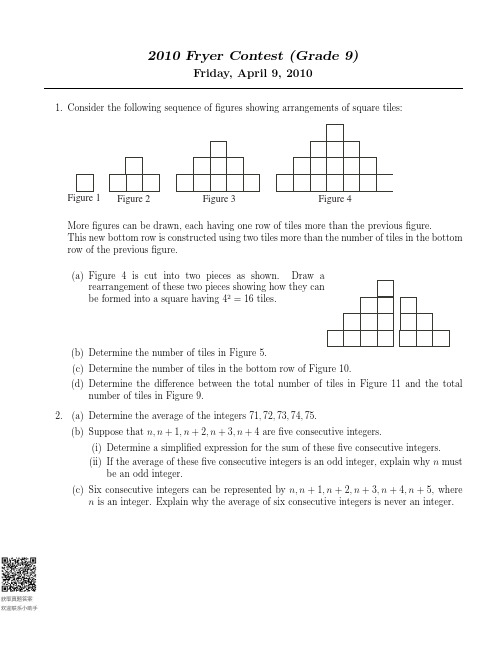
2010Fryer Contest(Grade9)Friday,April9,20101.Consider the following sequence ofﬁgures showing arrangements of square tiles:Figure 1Figure 2Figure 3Figure 4Moreﬁgures can be drawn,each having one row of tiles more than the previousﬁgure.This new bottom row is constructed using two tiles more than the number of tiles in the bottom row of the previousﬁgure.(a)Figure4is cut into two pieces as shown.Draw arearrangement of these two pieces showing how they canbe formed into a square having42=16tiles.(b)Determine the number of tiles in Figure5.(c)Determine the number of tiles in the bottom row of Figure10.(d)Determine the diﬀerence between the total number of tiles in Figure11and the totalnumber of tiles in Figure9.2.(a)Determine the average of the integers71,72,73,74,75.(b)Suppose that n,n+1,n+2,n+3,n+4areﬁve consecutive integers.(i)Determine a simpliﬁed expression for the sum of theseﬁve consecutive integers.(ii)If the average of theseﬁve consecutive integers is an odd integer,explain why n must be an odd integer.(c)Six consecutive integers can be represented by n,n+1,n+2,n+3,n+4,n+5,wheren is an integer.Explain why the average of six consecutive integers is never an integer.2010Fryer Contest Page23.Train1is travelling from Amville to Battonat a constant speed.Train2is travelling from Batton to Amville at a constantspeed.Train 1Train 2(a)Train1travels at60km/h and travels23of the distance to Batton in9hours.Determine the distance from Amville to Batton.(b)Train2travels23of the distance to Amville in6hours.How fast is the train going?(c)Train2started its trip312hours after Train1started its trip.Both trains arrived atCuford at9:00p.m.What time did Train1leave Amville?4.A palindrome is a positive integer that is the same when read forwards or backwards.Forexample,three palindromes are7,121and7739377.(a)Determine the number of palindromes less than1000.(b)Determine the number of palindromes with7digits.(c)If the palindromes in part(b)are written in increasing order,determine the2125thpalindrome in the list.(d)Determine the number of six-digit palindromes that are divisible by91.。

2011滑铁卢竞赛试题答案

1.(a)Since (x +1)+(x +2)+(x +3)=8+9+10,then 3x +6=27or 3x =21and so x =7.(b)Since 25+√x =6,then squaring both sides gives 25+√x =36or √x =11.Since √x =11,then squaring both sides again,we obtain x =112=121.Checking, 25+√121=√25+11=√36=6,as required.(c)Since (a,2)is the point of intersection of the lines with equations y =2x −4and y =x +k ,then the coordinates of this point must satisfy both equations.Using the ﬁrst equation,2=2a −4or 2a =6or a =3.Since the coordinates of the point (3,2)satisfy the equation y =x +k ,then 2=3+k or k =−1.2.(a)Since the side length of the original square is 3and an equilateral triangle of side length 1is removed from the middle of each side,then each of the two remaining pieces of each side of the square has length 1.Also,each of the two sides of each of the equilateral triangles that are shown has length 1.1111Therefore,each of the 16line segments in the ﬁgure has length 1,and so the perimeter of the ﬁgure is 16.(b)Since DC =DB ,then CDB is isosceles and ∠DBC =∠DCB =15◦.Thus,∠CDB =180◦−∠DBC −∠DCB =150◦.Since the angles around a point add to 360◦,then∠ADC =360◦−∠ADB −∠CDB =360◦−130◦−150◦=80◦.(c)By the Pythagorean Theorem in EAD ,we have EA 2+AD 2=ED 2or 122+AD 2=132,and so AD =√169−144=5,since AD >0.By the Pythagorean Theorem in ACD ,we have AC 2+CD 2=AD 2or AC 2+42=52,and so AC =√25−16=3,since AC >0.(We could also have determined the lengths of AD and AC by recognizing 3-4-5and 5-12-13right-angled triangles.)By the Pythagorean Theorem in ABC ,we have AB 2+BC 2=AC 2or AB 2+22=32,and so AB =√9−4=√5,since AB >0.3.(a)Solution 1Since we want to make 15−y x as large as possible,then we want to subtract as little as possible from 15.In other words,we want to make y x as small as possible.To make a fraction with positive numerator and denominator as small as possible,wemake the numerator as small as possible and the denominator as large as possible.Since 2≤x ≤5and 10≤y ≤20,then we make x =5and y =10.Therefore,the maximum value of 15−y x is 15−105=13.Solution2Since y is positive and2≤x≤5,then15−yx≤15−y5for any x with2≤x≤5andpositive y.Since10≤y≤20,then15−y5≤15−105for any y with10≤y≤20.Therefore,for any x and y in these ranges,15−yx≤15−105=13,and so the maximumpossible value is13(which occurs when x=5and y=10).(b)Solution1First,we add the two given equations to obtain(f(x)+g(x))+(f(x)−g(x))=(3x+5)+(5x+7)or2f(x)=8x+12which gives f(x)=4x+6.Since f(x)+g(x)=3x+5,then g(x)=3x+5−f(x)=3x+5−(4x+6)=−x−1.(We could alsoﬁnd g(x)by subtracting the two given equations or by using the second of the given equations.)Since f(x)=4x+6,then f(2)=14.Since g(x)=−x−1,then g(2)=−3.Therefore,2f(2)g(2)=2×14×(−3)=−84.Solution2Since the two given equations are true for all values of x,then we can substitute x=2to obtainf(2)+g(2)=11f(2)−g(2)=17Next,we add these two equations to obtain2f(2)=28or f(2)=14.Since f(2)+g(2)=11,then g(2)=11−f(2)=11−14=−3.(We could alsoﬁnd g(2)by subtracting the two equations above or by using the second of these equations.)Therefore,2f(2)g(2)=2×14×(−3)=−84.4.(a)We consider choosing the three numbers all at once.We list the possible sets of three numbers that can be chosen:{1,2,3}{1,2,4}{1,2,5}{1,3,4}{1,3,5}{1,4,5}{2,3,4}{2,3,5}{2,4,5}{3,4,5} We have listed each in increasing order because once the numbers are chosen,we arrange them in increasing order.There are10sets of three numbers that can be chosen.Of these10,the4sequences1,2,3and1,3,5and2,3,4and3,4,5are arithmetic sequences.Therefore,the probability that the resulting sequence is an arithmetic sequence is410or25.(b)Solution 1Join B to D .AConsider CBD .Since CB =CD ,then ∠CBD =∠CDB =12(180◦−∠BCD )=12(180◦−60◦)=60◦.Therefore, BCD is equilateral,and so BD =BC =CD =6.Consider DBA .Note that ∠DBA =90◦−∠CBD =90◦−60◦=30◦.Since BD =BA =6,then ∠BDA =∠BAD =12(180◦−∠DBA )=12(180◦−30◦)=75◦.We calculate the length of AD .Method 1By the Sine Law in DBA ,we have AD sin(∠DBA )=BA sin(∠BDA ).Therefore,AD =6sin(30◦)sin(75◦)=6×12sin(75◦)=3sin(75◦).Method 2If we drop a perpendicular from B to P on AD ,then P is the midpoint of AD since BDA is isosceles.Thus,AD =2AP .Also,BP bisects ∠DBA ,so ∠ABP =15◦.Now,AP =BA sin(∠ABP )=6sin(15◦).Therefore,AD =2AP =12sin(15◦).Method 3By the Cosine Law in DBA ,AD 2=AB 2+BD 2−2(AB )(BD )cos(∠ABD )=62+62−2(6)(6)cos(30◦)=72−72(√32)=72−36√3Therefore,AD = 36(2−√3)=6 2−√3since AD >0.Solution 2Drop perpendiculars from D to Q on BC and from D to R on BA .AThen CQ =CD cos(∠DCQ )=6cos(60◦)=6×12=3.Also,DQ =CD sin(∠DCQ )=6sin(60◦)=6×√32=3√3.Since BC =6,then BQ =BC −CQ =6−3=3.Now quadrilateral BQDR has three right angles,so it must have a fourth right angle and so must be a rectangle.Thus,RD =BQ =3and RB =DQ =3√3.Since AB =6,then AR =AB −RB =6−3√3.Since ARD is right-angled at R ,then using the Pythagorean Theorem and the fact that AD >0,we obtain AD =√RD 2+AR 2= 32+(6−3√3)2= 9+36−36√3+27= 72−36√3which we can rewrite as AD = 36(2−√3)=6 2−√3.5.(a)Let n be the original number and N be the number when the digits are reversed.Sincewe are looking for the largest value of n ,we assume that n >0.Since we want N to be 75%larger than n ,then N should be 175%of n ,or N =74n .Suppose that the tens digit of n is a and the units digit of n is b .Then n =10a +b .Also,the tens digit of N is b and the units digit of N is a ,so N =10b +a .We want 10b +a =74(10a +b )or 4(10b +a )=7(10a +b )or 40b +4a =70a +7b or 33b =66a ,and so b =2a .This tells us that that any two-digit number n =10a +b with b =2a has the required property.Since both a and b are digits then b <10and so a <5,which means that the possible values of n are 12,24,36,and 48.The largest of these numbers is 48.(b)We “complete the rectangle”by drawing a horizontal line through C which meets they -axis at P and the vertical line through B at Q .x A (0,Since C has y -coordinate 5,then P has y -coordinate 5;thus the coordinates of P are (0,5).Since B has x -coordinate 4,then Q has x -coordinate 4.Since C has y -coordinate 5,then Q has y -coordinate 5.Therefore,the coordinates of Q are (4,5),and so rectangle OP QB is 4by 5and so has area 4×5=20.Now rectangle OP QB is made up of four smaller triangles,and so the sum of the areas of these triangles must be 20.Let us examine each of these triangles:• ABC has area 8(given information)• AOB is right-angled at O ,has height AO =3and base OB =4,and so has area 12×4×3=6.• AP C is right-angled at P ,has height AP =5−3=2and base P C =k −0=k ,and so has area 1×k ×2=k .• CQB is right-angled at Q ,has height QB =5−0=5and base CQ =4−k ,andso has area 12×(4−k )×5=10−52k .Since the sum of the areas of these triangles is 20,then 8+6+k +10−52k =20or 4=32k and so k =83.6.(a)Solution 1Suppose that the distance from point A to point B is d km.Suppose also that r c is the speed at which Serge travels while not paddling (i.e.being carried by just the current),that r p is the speed at which Serge travels with no current (i.e.just from his paddling),and r p +c his speed when being moved by both his paddling and the current.It takes Serge 18minutes to travel from A to B while paddling with the current.Thus,r p +c =d 18km/min.It takes Serge 30minutes to travel from A to B with just the current.Thus,r c =d 30km/min.But r p =r p +c −r c =d 18−d 30=5d 90−3d 90=2d 90=d 45km/min.Since Serge can paddle the d km from A to B at a speed of d 45km/min,then it takes him 45minutes to paddle from A to B with no current.Solution 2Suppose that the distance from point A to point B is d km,the speed of the current of the river is r km/h,and the speed that Serge can paddle is s km/h.Since the current can carry Serge from A to B in 30minutes (or 12h),then d r =12.When Serge paddles with the current,his speed equals his paddling speed plus the speed of the current,or (s +r )km/h.Since Serge can paddle with the current from A to B in 18minutes (or 310h),then d r +s =310.The time to paddle from A to B with no current would be d s h.Since d r =12,then r d =2.Since d r +s =310,then r +s d =103.Therefore,s d =r +s d −r d =103−2=43.Thus,d s =34,and so it would take Serge 34of an hour,or 45minutes,to paddle from A to B with no current.Solution 3Suppose that the distance from point A to point B is d km,the speed of the current of the river is r km/h,and the speed that Serge can paddle is s km/h.Since the current can carry Serge from A to B in 30minutes (or 12h),then d r =12or d =1r .When Serge paddles with the current,his speed equals his paddling speed plus the speed of the current,or (s +r )km/h.Since Serge can paddle with the current from A to B in 18minutes (or 310h),then d r +s =310or d =310(r +s ).Since d =12r and d =310(r +s ),then 12r =310(r +s )or 5r =3r +3s and so s =23r .To travel from A to B with no current,the time in hours that it takes is d s =12r 2r =34,or 45minutes.(b)First,we note that a =0.(If a =0,then the “parabola”y =a (x −2)(x −6)is actuallythe horizontal line y =0which intersects the square all along OR .)Second,we note that,regardless of the value of a =0,the parabola has x -intercepts 2and 6,and so intersects the x -axis at (2,0)and (6,0),which we call K (2,0)and L (6,0).This gives KL =4.Third,we note that since the x -intercepts of the parabola are 2and 6,then the axis ofsymmetry of the parabola has equation x =12(2+6)=4.Since the axis of symmetry of the parabola is a vertical line of symmetry,then if theparabola intersects the two vertical sides of the square,it will intersect these at the same height,and if the parabola intersects the top side of the square,it will intersect it at two points that are symmetrical about the vertical line x =4.Fourth,we recall that a trapezoid with parallel sides of lengths a and b and height h hasarea 12h (a +b ).We now examine three cases.Case1:a<0Here,the parabola opens downwards.Since the parabola intersects the square at four points,it must intersect P Q at points M and N.(The parabola cannot intersect the vertical sides of the square since it gets “narrower”towards the vertex.)xx =4Since the parabola opens downwards,then MN<KL=4.Since the height of the trapezoid equals the height of the square(or8),then the area of the trapezoid is1h(KL+MN)which is less than1(8)(4+4)=32.But the area of the trapezoid must be36,so this case is not possible.Case2:a>0;M and N on P QWe have the following conﬁguration:xx =4Here,the height of the trapezoid is8,KL=4,and M and N are symmetric about x=4.Since the area of the trapezoid is36,then12h(KL+MN)=36or12(8)(4+MN)=36or4+MN=9or MN=5.Thus,M and N are each52units from x=4,and so N has coordinates(32,8).Since this point lies on the parabola with equation y=a(x−2)(x−6),then8=a(32−2)(32−6)or8=a(−12)(−92)or8=94a or a=329.Case3:a>0;M and N on QR and P Oxx =4Here,KL=4,MN=8,and M and N have the same y-coordinate.Since the area of the trapezoid is36,then12h(KL+MN)=36or12h(4+8)=36or6h=36or h=6.Thus,N has coordinates(0,6).Since this point lies on the parabola with equation y=a(x−2)(x−6),then 6=a(0−2)(0−6)or6=12a or a=12.Therefore,the possible values of a are329and12.7.(a)Solution1Consider a population of100people,each of whom is75years old and who behave ac-cording to the probabilities given in the question.Each of the original100people has a50%chance of living at least another10years,so there will be50%×100=50of these people alive at age85.Each of the original100people has a20%chance of living at least another15years,so there will be20%×100=20of these people alive at age90.Since there is a25%(or14)chance that an80year old person will live at least another10years(that is,to age90),then there should be4times as many of these people alive at age80than at age90.Since there are20people alive at age90,then there are4×20=80of the original100 people alive at age80.In summary,of the initial100people of age75,there are80alive at age80,50alive at age85,and20people alive at age90.Because50of the80people alive at age80are still alive at age85,then the probability that an80year old person will live at least5more years(that is,to age85)is50=5,or 62.5%.Solution2Suppose that the probability that a75year old person lives to80is p,the probability that an80year old person lives to85is q,and the probability that an85year old person lives to90is r.We want to the determine the value of q.For a75year old person to live at least another10years,they must live another5years (to age80)and then another5years(to age85).The probability of this is equal to pq. We are told in the question that this is equal to50%or0.5.Therefore,pq=0.5.For a75year old person to live at least another15years,they must live another5years (to age80),then another5years(to age85),and then another5years(to age90).The probability of this is equal to pqr.We are told in the question that this is equal to20% or0.2.Therefore,pqr=0.2Similarly,since the probability that an80year old person will live another10years is25%,then qr=0.25.Since pqr=0.2and pq=0.5,then r=pqrpq=0.20.5=0.4.Since qr=0.25and r=0.4,then q=qrr=0.250.4=0.625.Therefore,the probability that an80year old man will live at least another5years is0.625,or62.5%.(b)Using logarithm rules,the given equation is equivalent to22log10x=3(2·2log10x)+16or(2log10x)2=6·2log10x+16.Set u=2log10x.Then the equation becomes u2=6u+16or u2−6u−16=0.Factoring,we obtain(u−8)(u+2)=0and so u=8or u=−2.Since2a>0for any real number a,then u>0and so we can reject the possibility that u=−2.Thus,u=2log10x=8which means that log10x=3.Therefore,x=1000.8.(a)First,we determine theﬁrst entry in the50th row.Since theﬁrst column is an arithmetic sequence with common diﬀerence3,then the50th entry in theﬁrst column(theﬁrst entry in the50th row)is4+49(3)=4+147=151.Second,we determine the common diﬀerence in the50th row by determining the second entry in the50th row.Since the second column is an arithmetic sequence with common diﬀerence5,then the 50th entry in the second column(that is,the second entry in the50th row)is7+49(5) or7+245=252.Therefore,the common diﬀerence in the50th row must be252−151=101.Thus,the40th entry in the50th row(that is,the number in the50th row and the40th column)is151+39(101)=151+3939=4090.(b)We follow the same procedure as in(a).First,we determine theﬁrst entry in the R th row.Since theﬁrst column is an arithmetic sequence with common diﬀerence3,then the R th entry in theﬁrst column(that is,theﬁrst entry in the R th row)is4+(R−1)(3)or 4+3R−3=3R+1.Second,we determine the common diﬀerence in the R th row by determining the second entry in the R th row.Since the second column is an arithmetic sequence with common diﬀerence5,then the R th entry in the second column(that is,the second entry in the R th row)is7+(R−1)(5) or7+5R−5=5R+2.Therefore,the common diﬀerence in the R th row must be(5R+2)−(3R+1)=2R+1.Thus,the C th entry in the R th row(that is,the number in the R th row and the C th column)is3R+1+(C−1)(2R+1)=3R+1+2RC+C−2R−1=2RC+R+C(c)Suppose that N is an entry in the table,say in the R th row and C th column.From(b),then N=2RC+R+C and so2N+1=4RC+2R+2C+1.Now4RC+2R+2C+1=2R(2C+1)+2C+1=(2R+1)(2C+1).Since R and C are integers with R≥1and C≥1,then2R+1and2C+1are each integers that are at least3.Therefore,2N+1=(2R+1)(2C+1)must be composite,since it is the product of two integers that are each greater than1.9.(a)If n=2011,then8n−7=16081and so √8n−7≈126.81.Thus,1+√8n−72≈1+126.812≈63.9.Therefore,g(2011)=2(2011)+1+8(2011)−72=4022+ 63.9 =4022+63=4085.(b)To determine a value of n for which f(n)=100,we need to solve the equation2n−1+√8n−72=100(∗)Weﬁrst solve the equation2x−1+√8x−72=100(∗∗)because the left sides of(∗)and(∗∗)do not diﬀer by much and so the solutions are likely close together.We will try integers n in(∗)that are close to the solutions to(∗∗). Manipulating(∗∗),we obtain4x−(1+√8x−7)=2004x−201=√8x−7(4x−201)2=8x−716x2−1608x+40401=8x−716x2−1616x+40408=02x2−202x+5051=0By the quadratic formula,x=202±2022−4(2)(5051)2(2)=202±√3964=101±√992and so x≈55.47or x≈45.53.We try n=55,which is close to55.47:f(55)=2(55)−1+8(55)−72=110−1+√4332Since √433≈20.8,then1+√4332≈10.9,which gives1+√4332=10.Thus,f(55)=110−10=100.Therefore,a value of n for which f(n)=100is n=55.(c)We want to show that each positive integer m is in the range of f or the range of g ,butnot both.To do this,we ﬁrst try to better understand the “complicated”term of each of the func-tions –that is,the term involving the greatest integer function.In particular,we start witha positive integer k ≥1and try to determine the positive integers n that give 1+√8n −72 =k .By deﬁnition of the greatest integer function,the equation 1+√8n −72 =k is equiv-alent to the inequality k ≤1+√8n −72<k +1,from which we obtain the following set of equivalent inequalities 2k ≤1+√8n −7<2k +22k −1≤√8n −7<2k +14k 2−4k +1≤8n −7<4k 2+4k +14k 2−4k +8≤8n <4k 2+4k +812(k 2−k )+1≤n <12(k 2+k )+1If we deﬁne T k =1k (k +1)=1(k 2+k )to be the k th triangular number for k ≥0,thenT k −1=12(k −1)(k )=12(k 2−k ).Therefore, 1+√8n −72 =k for T k −1+1≤n <T k +1.Since n is an integer,then 1+√8n −72=k is true for T k −1+1≤n ≤T k .When k =1,this interval is T 0+1≤n ≤T 1(or 1≤n ≤1).When k =2,this interval is T 1+1≤n ≤T 2(or 2≤n ≤3).When k =3,this interval is T 2+1≤n ≤T 3(or 4≤n ≤6).As k ranges over all positive integers,these intervals include every positive integer n and do not overlap.Therefore,we can determine the range of each of the functions f and g by examining the values f (n )and g (n )when n is in these intervals.For each non-negative integer k ,deﬁne R k to be the set of integers greater than k 2and less than or equal to (k +1)2.Thus,R k ={k 2+1,k 2+2,...,k 2+2k,k 2+2k +1}.For example,R 0={1},R 1={2,3,4},R 2={5,6,7,8,9},and so on.Every positive integer occurs in exactly one of these sets.Also,for each non-negative integer k deﬁne S k ={k 2+2,k 2+4,...,k 2+2k }and deﬁne Q k ={k 2+1,k 2+3,...,k 2+2k +1}.For example,S 0={},S 1={3},S 2={6,8},Q 0={1},Q 1={2,4},Q 2={5,7,9},and so on.Note that R k =Q k ∪S k so every positive integer occurs in exactly one Q k or in exactly one S k ,and that these sets do not overlap since no two S k ’s overlap and no two Q k ’s overlap and no Q k overlaps with an S k .We determine the range of the function g ﬁrst.For T k −1+1≤n ≤T k ,we have 1+√8n −72=k and so 2T k −1+2≤2n ≤2T k 2T k −1+2+k ≤2n + 1+√8n −72 ≤2T k +k k 2−k +2+k ≤g (n )≤k 2+k +k k 2+2≤g (n )≤k 2+2kNote that when n is in this interval and increases by 1,then the 2n term causes the value of g (n )to increase by 2.Therefore,for the values of n in this interval,g (n )takes precisely the values k 2+2,k 2+4,k 2+6,...,k 2+2k .In other words,the range of g over this interval of its domain is precisely the set S k .As k ranges over all positive integers (that is,as these intervals cover the domain of g ),this tells us that the range of g is precisely the integers in the sets S 1,S 2,S 3,....(We could also include S 0in this list since it is the empty set.)We note next that f (1)=2− 1+√8−72 =1,the only element of Q 0.For k ≥1and T k +1≤n ≤T k +1,we have 1+√8n −72=k +1and so 2T k +2≤2n ≤2T k +12T k +2−(k +1)≤2n − 1+√8n −72 ≤2T k +1−(k +1)k 2+k +2−k −1≤f (n )≤(k +1)(k +2)−k −1k 2+1≤f (n )≤k 2+2k +1Note that when n is in this interval and increases by 1,then the 2n term causes the value of f (n )to increase by 2.Therefore,for the values of n in this interval,f (n )takes precisely the values k 2+1,k 2+3,k 2+5,...,k 2+2k +1.In other words,the range of f over this interval of its domain is precisely the set Q k .As k ranges over all positive integers (that is,as these intervals cover the domain of f ),this tells us that the range of f is precisely the integers in the sets Q 0,Q 1,Q 2,....Therefore,the range of f is the set of elements in the sets Q 0,Q 1,Q 2,...and the range of g is the set of elements in the sets S 0,S 1,S 2,....These ranges include every positive integer and do not overlap.10.(a)Suppose that ∠KAB =θ.Since ∠KAC =2∠KAB ,then ∠KAC =2θand ∠BAC =∠KAC +∠KAB =3θ.Since 3∠ABC =2∠BAC ,then ∠ABC =23×3θ=2θ.Since ∠AKC is exterior to AKB ,then ∠AKC =∠KAB +∠ABC =3θ.This gives the following conﬁguration:BNow CAK is similar to CBA since the triangles have a common angle at C and ∠CAK =∠CBA .Therefore,AKBA=CACBordc=baand so d=bca.Also,CKCA=CACBora−xb=baand so a−x=b2aor x=a−b2a=a2−b2a,as required.(b)From(a),bc=ad and a2−b2=ax and so we obtainLS=(a2−b2)(a2−b2+ac)=(ax)(ax+ac)=a2x(x+c) andRS=b2c2=(bc)2=(ad)2=a2d2In order to show that LS=RS,we need to show that x(x+c)=d2(since a>0).Method1:Use the Sine LawFirst,we derive a formula for sin3θwhich we will need in this solution:sin3θ=sin(2θ+θ)=sin2θcosθ+cos2θsinθ=2sinθcos2θ+(1−2sin2θ)sinθ=2sinθ(1−sin2θ)+(1−2sin2θ)sinθ=3sinθ−4sin3θSince∠AKB=180◦−∠KAB−∠KBA=180◦−3θ,then using the Sine Law in AKB givesx sinθ=dsin2θ=csin(180◦−3θ)Since sin(180◦−X)=sin X,then sin(180◦−3θ)=sin3θ,and so x=d sinθsin2θandc=d sin3θsin2θ.This givesx(x+c)=d sinθsin2θd sinθsin2θ+d sin3θsin2θ=d2sinθsin22θ(sinθ+sin3θ)=d2sinθsin22θ(sinθ+3sinθ−4sin3θ)=d2sinθsin22θ(4sinθ−4sin3θ)=4d2sin2θsin22θ(1−sin2θ)=4d2sin2θcos2θsin22θ=4d2sin2θcos2θ(2sinθcosθ)2=4d2sin2θcos2θ4sin2θcos2θ=d2as required.We could have instead used the formula sin A +sin B =2sinA +B 2 cos A −B2 toshow that sin 3θ+sin θ=2sin 2θcos θ,from which sin θ(sin 3θ+sin θ)=sin θ(2sin 2θcos θ)=2sin θcos θsin 2θ=sin 22θMethod 2:Extend ABExtend AB to E so that BE =BK =x and join KE .ENow KBE is isosceles with ∠BKE =∠KEB .Since ∠KBA is the exterior angle of KBE ,then ∠KBA =2∠KEB =2θ.Thus,∠KEB =∠BKE =θ.But this also tells us that ∠KAE =∠KEA =θ.Thus, KAE is isosceles and so KE =KA =d.ESo KAE is similar to BKE ,since each has two angles equal to θ.Thus,KA BK =AE KE or d x =c +x dand so d 2=x (x +c ),as required.Method 3:Use the Cosine Law and the Sine LawWe apply the Cosine Law in AKB to obtainAK 2=BK 2+BA 2−2(BA )(BK )cos(∠KBA )d 2=x 2+c 2−2cx cos(2θ)d 2=x 2+c 2−2cx (2cos 2θ−1)Using the Sine Law in AKB ,we get x sin θ=d sin 2θor sin 2θsin θ=d x or 2sin θcos θsin θ=d x and so cos θ=d 2x.Combining these two equations,d2=x2+c2−2cx2d24x2−1d2=x2+c2−cd2x+2cxd2+cd2x=x2+2cx+c2d2+cd2x=(x+c)2xd2+cd2=x(x+c)2d2(x+c)=x(x+c)2d2=x(x+c)as required(since x+c=0).(c)Solution1Our goal is toﬁnd a triple of positive integers that satisfy the equation in(b)and are the side lengths of a triangle.First,we note that if(A,B,C)is a triple of real numbers that satisﬁes the equation in(b)and k is another real number,then the triple(kA,kB,kC)also satisﬁes the equationfrom(b),since(k2A2−k2B2)(k2A2−k2B2+kAkC)=k4(A2−B2)(A2−B2+AC)=k4(B2C2)=(kB)2(kC)2 Therefore,we start by trying toﬁnd a triple(a,b,c)of rational numbers that satisﬁes the equation in(b)and forms a triangle,and then“scale up”this triple to form a triple (ka,kb,kc)of integers.To do this,we rewrite the equation from(b)as a quadratic equation in c and solve for c using the quadratic formula.Partially expanding the left side from(b),we obtain(a2−b2)(a2−b2)+ac(a2−b2)=b2c2which we rearrange to obtainb2c2−c(a(a2−b2))−(a2−b2)2=0By the quadratic formula,c=a(a2−b2)±a2(a2−b2)2+4b2(a2−b2)22b2=a(a2−b2)±(a2−b2)2(a2+4b2)2b2Since∠BAC>∠ABC,then a>b and so a2−b2>0,which givesc=a(a2−b2)±(a2−b2)√a2+4b22b2=(a2−b2)2b2(a±√a2+4b2)Since a2+4b2>0,then √a2+4b2>a,so the positive root isc=(a2−b2)2b2(a+a2+(2b)2)We try to ﬁnd integers a and b that give a rational value for c .We will then check to see if this triple (a,b,c )forms the side lengths of a triangle,and then eventually scale these up to get integer values.One way for the value of c to be rational (and in fact the only way)is for a 2+(2b )2to be an integer,or for a and 2b to be the legs of a Pythagorean triple.Since √32+42is an integer,then we try a =3and b =2,which givesc =(32−22)2·22(3+√32+42)=5and so (a,b,c )=(3,2,5).Unfortunately,these lengths do not form a triangle,since 3+2=5.(The Triangle Inequality tells us that three positive real numbers a ,b and c form a triangle if and only if a +b >c and a +c >b and b +c >a .)We can continue to try small Pythagorean triples.Now 152+82=172,but a =15and b =4do not give a value of c that forms a triangle with a and b .However,162+302=342,so we can try a =16and b =15which givesc =(162−152)2·152(16+√162+302)=31450(16+34)=319Now the lengths (a,b,c )=(16,15,319)do form the sides of a triangle since a +b >c and a +c >b and b +c >a .Since these values satisfy the equation from (b),then we can scale them up by a factor of k =9to obtain the triple (144,135,31)which satisﬁes the equation from (b)and are the side lengths of a triangle.(Using other Pythagorean triples,we could obtain other triples of integers that work.)Solution 2We note that the equation in (b)involves only a ,b and c and so appears to depend only on the relationship between the angles ∠CAB and ∠CBA in ABC .Using this premise,we use ABC ,remove the line segment AK and draw the altitude CF .CBA 3θ2θb aa c os 2θbc os 3θF Because we are only looking for one triple that works,we can make a number of assump-tions that may or may not be true in general for such a triangle,but which will help us ﬁnd an example.We assume that 3θand 2θare both acute angles;that is,we assume that θ<30◦.In ABC ,we have AF =b cos 3θ,BF =a cos 2θ,and CF =b sin 3θ=a sin 2θ.Note also that c =b cos 3θ+a cos 2θ.One way to ﬁnd the integers a,b,c that we require is to look for integers a and b and an angle θwith the properties that b cos 3θand a cos 2θare integers and b sin 3θ=a sin 2θ.Using trigonometric formulae,sin 2θ=2sin θcos θcos 2θ=2cos 2θ−1sin 3θ=3sin θ−4sin 3θ(from the calculation in (a),Solution 1,Method 1)cos 3θ=cos(2θ+θ)=cos 2θcos θ−sin 2θsin θ=(2cos 2θ−1)cos θ−2sin 2θcos θ=(2cos 2θ−1)cos θ−2(1−cos 2θ)cos θ=4cos 3θ−3cos θSo we can try to ﬁnd an angle θ<30◦with cos θa rational number and then integers a and b that make b sin 3θ=a sin 2θand ensure that b cos 3θand a cos 2θare integers.Since we are assuming that θ<30◦,then cos θ>√32≈0.866.The rational number with smallest denominator that is larger than √32is 78,so we try the acute angle θwith cos θ=7.In this case,sin θ=√1−cos 2θ=√158,and sosin 2θ=2sin θcos θ=2×78×√158=7√1532cos 2θ=2cos 2θ−1=2×4964−1=1732sin 3θ=3sin θ−4sin 3θ=3×√158−4×15√15512=33√15128cos 3θ=4cos 3θ−3cos θ=4×343512−3×78=7128To have b sin 3θ=a sin 2θ,we need 33√15128b =7√1532a or 33b =28a .To ensure that b cos 3θand a cos 2θare integers,we need 7128b and 1732a to be integers,andso a must be divisible by 32and b must be divisible by 128.The integers a =33and b =28satisfy the equation 33b =28a .Multiplying each by 32gives a =1056and b =896which satisfy the equation 33b =28a and now have the property that b is divisible by 128(with quotient 7)and a is divisible by 32(with quotient 33).With these values of a and b ,we obtain c =b cos 3θ+a cos 2θ=896×7128+1056×1732=610.We can then check that the triple (a,b,c )=(1056,896,610)satisﬁes the equation from(b),as required.As in our discussion in Solution 1,each element of this triple can be divided by 2to obtain the “smaller”triple (a,b,c )=(528,448,305)that satisﬁes the equation too.Using other values for cos θand integers a and b ,we could obtain other triples (a,b,c )of integers that work.。

2010PascalSolution滑铁卢竞赛题答案

1.In cents,theﬁve given choices are50,90,95,101,and115cents.The diﬀerences between each of these and$1.00(or100cents),in cents,are100−50=50100−90=10100−95=5101−100=1115−100=15 The diﬀerence between$1.01and$1.00is the smallest(1cent),so$1.01is closest to$1.00.Answer:(D) ing the correct order of operations,(20−16)×(12+8)4=4×204=804=20Answer:(C)3.We divide the750mL ofﬂour into portions of250mL.We do this by calculating750÷250=3.Therefore,750mL is three portions of250mL.Since50mL of milk is required for each250mL ofﬂour,then3×50=150mL of milk is required in total.Answer:(C) 4.There are8ﬁgures in total.Of these,3are triangles.Therefore,the probability is3.Answer:(A) 5.We simplify the left side and express it as a fraction with numerator1:1 9+118=218+118=318=16Therefore,the number that replaces the is6.Answer:(C) 6.There are16horizontal segments on the perimeter.Each has length1,so the horizontalsegments contribute16to the perimeter.There are10vertical segments on the perimeter.Each has length1,so the vertical segments contribute10to the perimeter.Therefore,the perimeter is10+16=26.(We could arrive at this total instead by starting at aﬁxed point and travelling around the outside of theﬁgure counting the number of segments.)Answer:(E) 7.Since33=3×3×3=3×9=27,then√33+33+33=√27+27+27=√81=9Answer:(B)8.The diﬀerence between the two given numbers is7.62−7.46=0.16.This length of the number line is divided into8equal segments.The length of each of these segments is thus0.16÷8=0.02.Point P is three of these segments to the right of7.46.Thus,the number represented is7.46+3(0.02)=7.46+0.06=7.52.Answer:(E)9.A 12by 12grid of squares will have 11interior vertical lines and 11interior horizontal lines.(In the given 4by 4example,there are 3interior vertical lines and 3interior horizontal lines.)Each of the 11interior vertical lines intersects each of the 11interior horizontal lines and creates an interior intersection point.Thus,each interior vertical line accounts for 11intersection points.Therefore,the number of interior intersection points is 11×11=121.Answer:(B)10.Because the central angle for the interior sector “Less than 1hour”is 90◦,then the fraction of the students who do less than 1hour of homework per day is 90◦360◦=14.In other words,25%of the students do less than 1hour of homework per day.Therefore,100%−25%=75%of the students do at least 1hour of homework per day.Answer:(E)11.Solution 1Since there is more than 1four-legged table,then there are at least 2four-legged tables.Since there are 23legs in total,then there must be fewer than 6four-legged tables,since 6four-legged tables would have 6×4=24legs.Thus,there are between 2and 5four-legged tables.If there are 2four-legged tables,then these tables account for 2×4=8legs,leaving 23−8=15legs for the three-legged tables.Since 15is divisible by 3,then this must be the solution,so there are 15÷3=5three-legged tables.(We can check that if there are 3or 4four-legged tables,then the number of remaining legs is not divisible by 3,and if there are 5four-legged tables,then there is only 1three-legged table,which is not allowed.)Solution 2Since there is more than 1table of each type,then there are at least 2three-legged tables and 2four-legged tables.These tables account for 2(3)+2(4)=14legs.There are 23−14=9more legs that need to be accounted for.These must come from a combination of three-legged and four-legged tables.The only way to make 9from 3s and 4s is to use three 3s.Therefore,there are 2+3=5three-legged tables and 2four-legged tables.Answer:(E)12.Solution 1The total area of the rectangle is 3×4=12.The total area of the shaded regions equals the total area of the rectangle (12)minus the area of the unshaded region.The unshaded region is a triangle with base of length 1and height 4;the area of this region is 12(1)(4)=2.Therefore,the total area of the shaded regions is 12−2=10.Solution 2The shaded triangle on the left has base of length 2and height of length 4,so has an area of 12(2)(4)=4.The shaded triangle on the right has base of length3(at the top)and height of length4,sohas an area of12(3)(4)=6.Therefore,the total area of the shaded regions is4+6=10.Answer:(C)13.Since the ratio of boys to girls at Cayley H.S.is3:2,then33+2=35of the students at CayleyH.S.are boys.Thus,there are35(400)=12005=240boys at Cayley H.S.Since the ratio of boys to girls at Fermat C.I.is2:3,then22+3=25of the students at FermatC.I.are boys.Thus,there are25(600)=12005=240boys at Fermat C.I.There are400+600=1000students in total at the two schools.Of these,240+240=480are boys,and so the remaining1000−480=520students are girls.Therefore,the overall ratio of boys to girls is480:520=48:52=12:13.Answer:(B) 14.When the given net is folded,the face numbered5will be opposite the face numbered1.Therefore,the remaining four faces share an edge with the face numbered1,so the product of the numbers is2×3×4×6=144.Answer:(B)15.The percentage10%is equivalent to the fraction110.Therefore,t=110s,or s=10t.Answer:(D)16.Since the base of the folded box measures5cm by4cm,then the area of the base of the boxis5(4)=20cm2.Since the volume of the box is60cm3and the area of the base is20cm2,then the height of the box is60=3cm.Therefore,each of the four identical squares has side length3cm,because the edges of these squares form the vertical edges of thebox.Therefore,the rectangular sheet measures3+5+3=11cm by3+4+3=10cm,and so has area11(10)=110cm2.Answer:(B) 17.Solution1Since SUR is a straight line,then∠RUV=180◦−∠SUV=180◦−120◦=60◦.Since P W and QX are parallel,then∠RV W=∠V T X=112◦.Since UV W is a straight line,then∠RV U=180◦−∠RV W=180◦−112◦=68◦.Since the measures of the angles in a triangle add to180◦,then∠URV=180◦−∠RUV−∠RV U=180◦−60◦−68◦=52◦Solution2Since SUR is a straight line,then∠RUV=180◦−∠SUV=180◦−120◦=60◦.Since P W and QX are parallel,then∠RST=∠RUV=60◦.Since ST X is a straight line,then∠RT S=180◦−∠V T X=180◦−112◦=68◦.Since the measures of the angles in a triangle add to180◦,then∠URV=∠SRT=180◦−∠RST−∠RT S=180◦−60◦−68◦=52◦Answer:(A) 18.Solution1When Catherine adds30litres of gasoline,the tank goes from18full to34full.Since34−18=68−18=58,then58of the capacity of the tank is30litres.Thus,18of the capacity of the tank is30÷5=6litres.Also,the full capacity of the tank is8×6=48litres.Toﬁll the remaining14of the tank,Catherine must add an additional14×48=12litres of gas.Because each litre costs$1.38,it will cost12×$1.38=$16.56toﬁll the rest of the tank. Solution2Suppose that the capacity of the gas tank is x litres.Starting with1of a tank,30litres of gas makes the tank3full,so1x+30=3x or5x=30or x=48.The remaining capacity of the tank is14x=14(48)=12litres.At$1.38per litre,it will cost Catherine12×$1.38=$16.56toﬁll the rest of the tank.Answer:(C) 19.The area of a semi-circle with radius r is1πr2so the area of a semi-circle with diameter d is1 2π(12d)2=18πd2.The semicircles with diameters UV,V W,W X,XY,and Y Z each have equal diameter andthus equal area.The area of each of these semicircles is18π(52)=258π.The large semicircle has diameter UZ=5(5)=25,so has area18π(252)=6258π.The shaded area equals the area of the large semicircle,minus the area of two small semicircles, plus the area of three small semicircles,which equals the area of the large semicircle plus the area of one small semicircle.Therefore,the shaded area equals6258π+258π=6508π=3254π.Answer:(A)20.The sum of the odd numbers from5to21is5+7+9+11+13+15+17+19+21=117Therefore,the sum of the numbers in any row is one-third of this total,or39.This means as well that the sum of the numbers in any column or diagonal is also39.Since the numbers in the middle row add to39,then the number in the centre square is 39−9−17=13.Since the numbers in the middle column add to39,then the number in the middle square in the bottom row is39−5−13=21.591317x21Since the numbers in the bottom row add to39,then the number in the bottom right square is39−21−x=18−x.Since the numbers in the bottom left to top right diagonal add to39,then the number in the top right square is39−13−x=26−x.Since the numbers in the rightmost column add to39,then(26−x)+17+(18−x)=39or 61−2x=39or2x=22,and so x=11.We can complete the magic square as follows:195159131711217Answer:(B) 21.We label the numbers in the empty boxes as y and z,so the numbers in the boxes are thus8,y,z,26,x.Since the average of z and x is26,then x+z=2(26)=52or z=52−x.We rewrite the list as8,y,52−x,26,x.Since the average of26and y is52−x,then26+y=2(52−x)or y=104−26−2x=78−2x.We rewrite the list as8,78−2x,52−x,26,x.Since the average of8and52−x is78−2x,then8+(52−x)=2(78−2x)60−x=156−4x3x=96x=32Therefore,x=32.Answer:(D) 22.Since JKLM is a rectangle,then the angles at J and K are each90◦,so each of SJP andQKP is right-angled.By the Pythagorean Theorem in SJP,we haveSP2=JS2+JP2=522+392=2704+1521=4225Since SP>0,then SP=√4225=65.Since P QRS is a rhombus,then P Q=P S=65.By the Pythagorean Theorem in QKP,we haveKP2=P Q2−KQ2=652−252=4225−625=3600Since KP>0,then KP=√3600=60.(Instead of using the Pythagorean Theorem,we could note instead that SJP is a scaled-up version of a3-4-5right-angled triangle and that QKP is a scaled-up version of a5-12-13 right-angled triangle.This would allow us to use the known ratios of side lengths to calculate the missing side length.)Since KQ and P Z are parallel and P K and W Q are parallel,then P KQW is a rectangle,andso P W=KQ=25.Similarly,JP ZS is a rectangle and so P Z=JS=52.Thus,W Z=P Z−P W=52−25=27.Also,SY RM is a rectangle.Since JM and KL are parallel(JKLM is a rectangle),JK and ML are parallel,and P Q and SR are parallel(P QRS is a rhombus),then∠MSR=∠KQP and∠SRM=∠QP K.Since SMR and QKP have two equal angles,then their third angles must be equal too.Thus,the triangles have the same proportions.Since the hypotenuses of the triangles are equal, then the triangles must in fact be exactly the same size;that is,the lengths of the corresponding sides must be equal.(We say that SMR is congruent to QKP by“angle-side-angle”.) In particular,MR=KP=60.Thus,ZY=SY−SZ=MR−JP=60−39=21.Therefore,the perimeter of rectangle W XY Z is2(21)+2(27)=96.Answer:(D) 23.First,we note that2010=10(201)=2(5)(3)(67)and so20102=223252672.Consider N consecutive four-digit positive integers.For the product of these N integers to be divisible by20102,it must be the case that two diﬀerent integers are divisible by67(which would mean that there are at least68integers in the list)or one of the integers is divisible by672.Since we want to minimize N(and indeed because none of the answer choices is at least68), we look for a list of integers in which one is divisible by672=4489.Since the integers must all be four-digit integers,then the only multiples of4489the we must consider are4489and8978.First,we consider a list of N consecutive integers including4489.Since the product of these integers must have2factors of5and no single integer within10 of4489has a factor of25,then the list must include two integers that are multiples of5.To minimize the number of integers in the list,we try to include4485and4490.Thus our candidate list is4485,4486,4487,4488,4489,4490.The product of these integers includes2factors of67(in4489),2factors of5(in4485and 4490),2factors of2(in4486and4488),and2factors of3(since each of4485and4488is divisible by3).Thus,the product of these6integers is divisible by20102.Therefore,the shortest possible list including4489has length6.Next,we consider a list of N consecutive integers including8978.Here,there is a nearby integer containing2factors of5,namely8975.So we start with the list8975,8976,8977,8978and check to see if it has the required property.The product of these integers includes2factors of67(in8978),2factors of5(in8975),and2 factors of2(in8976).However,the only integer in this list divisible by3is8976,which has only1factor of3.To include a second factor of3,we must include a second multiple of3in the list.Thus,we extend the list by one number to8979.Therefore,the product of the numbers in the list8975,8976,8977,8978,8979is a multiple of 20102.The length of this list is5.Thus,the smallest possible value of N is5.(Note that a quick way to test if an integer is divisible by3is to add its digit and see if this total is divisible by3.For example,the sum of the digits of8979is33;since33is a multiple of3,then8979is a multiple of3.)Answer:(A)24.We label the terms x1,x2,x3,...,x2009,x2010.Suppose that S is the sum of the odd-numbered terms in the sequence;that is,S=x1+x3+x5+···+x2007+x2009We know that the sum of all of the terms is5307;that is,x1+x2+x3+···+x2009+x2010=5307Next,we pair up the terms:each odd-numbered term with the following even-numbered term.That is,we pair theﬁrst term with the second,the third term with the fourth,and so on,until we pair the2009th term with the2010th term.There are1005such pairs.In each pair,the even-numbered term is one bigger than the odd-numbered term.That is, x2−x1=1,x4−x3=1,and so on.Therefore,the sum of the even-numbered terms is1005greater than the sum of the odd-numbered terms.Thus,the sum of the even-numbered terms is S+1005.Since the sum of all of the terms equals the sum of the odd-numbered terms plus the sum of the even-numbered terms,then S+(S+1005)=5307or2S=4302or S=2151.Thus,the required sum is2151.Answer:(C) 25.Before we answer the given question,we determine the number of ways of choosing3objectsfrom5objects and the number of ways of choosing2objects from5objects.Consider5objects labelled B,C,D,E,F.The possible pairs are:BC,BD,BE,BF,CD,CE,CF,DE,DF,EF.There are10such pairs.The possible triples are:DEF,CEF,CDF,CDE,BEF,BDF,BDE,BCF,BCE,BCD.There are10such triples.(Can you see why there are the same number of pairs and triples?)Label the six teams A,B,C,D,E,F.We start by considering team A.Team A plays3games,so we must choose3of the remaining5teams for A to play.As we saw above,there are10ways to do this.Without loss of generality,we pick one of these sets of3teams for A to play,say A plays B,C and D.We keep track of everything by drawing diagrams,joining the teams that play each other witha line.Thus far,we haveAB C DThere are two possible cases now–either none of B,C and D play each other,or at least one pair of B,C,D plays each other.Case1:None of the teams that play A play each otherIn the conﬁguration above,each of B,C and D play two more games.They already play A and cannot play each other,so they must each play E and F.This givesAE FB C DNo further choices are possible.There are10possible schedules in this type of conﬁguration.These10combinations come from choosing the3teams that play A.Case2:Some of the teams that play A play each otherHere,at least one pair of the teams that play A play each other.Given the teams B,C and D playing A,there are3possible pairs(BC,BD,CD).We pick one of these pairs,say BC.(This gives10×3=30conﬁgurations so far.)AB C DIt is now not possible for B or C to also play D.If it was the case that C,say,played D,then we would have the conﬁgurationAB C DE FHere,A and C have each played3games and B and D have each played2games.Teams E and F are unaccounted for thus far.They cannot both play3games in this conﬁguration as the possible opponents for E are B,D and F,and the possible opponents for F are B,D and E,with the“B”and“D”possibilities only to be used once.A similar argument shows thatB cannot play D.Thus,B or C cannot also play D.So we have the conﬁgurationAB C DHere,A has played3games,B and C have each played2games,and D has played1game.B andC must play1more game and cannot playD or A.They must play E and F in some order.There are2possible ways to assign these games(BE and CF,or BF and CE.)This gives30×2=60conﬁgurations so far.Suppose that B plays E and C plays F.AB C DE FSo far,A,B and C each play3games and E,F and D each play1game.The only way to complete the conﬁguration is to join D,E and F.AB C DE FTherefore,there are60possible schedules in this case.In total,there are10+60=70possible schedules.Answer:(E)。

第十一届全国大学生数学竞赛(非数学类)试题

第十一届全国大学生数学竞赛(非数学类)试题参考解答及评分标准一、填空题（每小题6分）1. sin 014x x →=.解：sin sin 00x x x x x →→→=- sin 1/31/30022(e 1)1sin 1limlim 444422x x x x x x →→-=+-=⎛⎫⎛⎫ ⎪ ⎪⎝⎭⎝⎭. 2. 设隐函数()y y x =由方程22()y x y x -=所确定，则232ln ||dx y y C y x x=-+⎰. 解：令y tx =，则21(1)x t t =-，1(1)y t t =-，3223(1)tdx dt t t -+=-，这样，223332ln ||2ln ||dx t y ydt t t C C y t x x-+==-+=-+⎰⎰. 3. 定积分220(1sin )1cos x e x dx e xππ+=+⎰.解：222000(1sin )sin 1cos 1cos 1cos x xx e x e xdx dx de xx x πππ+=++++⎰⎰⎰ 2222200sin cos (1cos )+sin 1cos 1cos (1cos )xxxe xe x x x dx e dx x x x πππ+=+-+++⎰⎰2222000sin 1cos 1cos 1cos xxx e xe edx dx e x x x ππππ=+-=+++⎰⎰. 4. 已知22(,)323ydx xdy du x y x xy y -=-+，则1(,)()C 3x u x y y =-+. 解：22(,)323ydx xdy du x y x xy y -=-+21()233()3xd x yx x y y y ==--+（）.所以，1(,)()C 3x u x y y =-+.5. 设,,,0a b c μ>，曲面xyz μ=与曲面2222221x y z a b c ++=相切，则μ=.解：根据题意有：22x yz a λ=,22y xz b λ=，22zxy c λ=，以及 222x a μλ=，222y b μλ=，222z c μλ=，从而得：32228a b cλμ=，32μλ=，联立解得：μ=二、（14分）计算三重积分22d d d Ω+⎰⎰⎰xyzx y z x y，其中Ω是由曲面2222()2++=x y z xy 围成的区域在第一卦限部分.解：采用“球面坐标”计算，并利用对称性，得ππ3224222sin cos sin cos 2d d sin d sin I ρϕθθϕθϕρϕρρϕ=⎰⎰ -------5分ππ342002sin cos d sin cos d d θθθϕϕϕρρ=⎰⎰ππ3354202sin cos d sin cos d θθθϕϕϕ=⎰⎰ -------10分ππ354201sin 2d sin d(sin )4θθϕϕ=⎰⎰π3201121sin d 4848372t t ==⋅=⎰. -------14分三、（14分）设()f x 在[0,)+∞上可微，(0)0f =，且存在常数0A >，使得|()||()|f x A f x '≤在[0,)+∞上成立，试证明：在(0,)+∞上有()0f x ≡.证明：设01[0,]2x A ∈，使得01|()|max |()|[0,]2f x f x x A ⎧⎫=∈⎨⎬⎩⎭， -------5分 000011|()||(0)+()||()||()|22f x f f x A f x f x A ξ'=≤=，只有0|()|0f x =. 故当 1[0,]2x A∈时，()0f x ≡. -------12分递推可得，对所有的1[,]22k kx A A-∈，1,2,k =，均有()0f x ≡. -------14分四、（14分）计算积分2sin (cos sin )0sin I d e d ππθφφφθθ-=⎰⎰解：设球面 Σ:x 2+y 2+z 2=1, 由球面参数方程sin cos x θφ=，sin sin y θφ=，cos z θ=知sin dS d d θθφ=，所以，所求积分可化为第一型曲面积分I =∬e x−ydS Σ-------4分设平面P t :√2=t,−1≤t ≤1，其中t 为平面P t 被球面截下部分中心到原点距离.用平面P t 分割球面Σ，球面在平面P t ,P t+dt 之间的部分形如圆台外表面状，记为Σt,dt .被积函数在其上为 e x−y =e √2t . -------8分由于Σt,dt 半径为r t =√1−t 2，半径的增长率为 d√1−t 2=√1−t 2 就是 Σt,dt 上下底半径之差. 记圆台外表面斜高为ℎt ，则由微元法知 dt 2+(d √1−t 2)2=ℎt 2, 得到ℎt =√1−t 2 ，所以 Σt,dt 的面积为 dS =2πr t ℎt =2πdt, -------12分I =∫e √2t 1−12πdt =√2√2t |−11=√2π(e √2−e −√2). -------14分五、（14分）设()f x 是仅有正实根的多项式函数，满足 0()()n n n f x c x f x +∞='=-∑. 试证：0n c >，(0n ≥），极限lim n ()f x 的最小根. 证明：由f (x )为仅有正实根的多项式，不妨设()f x 的全部根为 0<a 1<a 2<⋯<a k ，这样，f (x )=A (x −a 1)r 1⋯(x −a k )r k ，其中 r i 为对应根a i 的重数 (i =1,⋯,k,r k ≥1). -------2分f ′(x )=Ar 1(x −a 1)r 1−1⋯(x −a k )r k +⋯+Ar k (x −a 1)r 1⋯(x −a k )r k −1，所以，f ′(x )=f (x )(r 1x−a 1+⋯+rkx−a k)，从而， −f ′(x)f(x)=r 1a 1∙11−xa 1+⋯+r k a k∙11−x a k.-------6分若|x |<a 1, 则 −f ′(x)f(x)=r 1a 1∙∑(xa1)n∞n=0+⋯+r k a k∙∑(xak)n∞n=0=∑(r 1a 1n+1+⋯+r k a kn+1)∞n=0x n .而 −f ′(x)f(x)=∑c n x n∞n=0，由幂级数的唯一性知c n =r 1a 1n+1+⋯+r kak n+1>0， ------9分c ncn+1=r 1a 1n+1+⋯+r k a kn+1r 1a 1n+2+⋯+r k a kn+2=a 1∙r 1+⋯+(a1a k)n+1r kr 1+⋯+(a 1a k)n+2r k.limn→∞c nc =a 1∙r 1+0+⋯+0r +0+⋯+0=a 1>0， limn→∞c n+1c =1a ， -----12分limn→∞1n ∙(ln c2c1+⋯+ln c n+1c n)=ln 1a 1,√c n n=elnc nn=elnc 1n +1n (ln c 2c 1+⋯+ln cn+1c n)→eln1a 1=1a 1.从而，lim√c nn=a 1,即f (x )的最小正根. -----14分六、（14分）设函数()f x 在[0, )+∞上具有连续导数，满足22223[3()]()2[1()]-'+=+x f x f x f x e ，且(0)1≤f .证明：存在常数0>M ，使得[0,)∈+∞x 时，恒有()≤f x M .证明：由于()0'>f x ，所以()f x 是[0, )+∞上的严格增函数，故+lim ()→∞=x f x L (有限或为+∞). 下面证明 ≠+∞L . -----2分记()=y f x ，将所给等式分离变量并积分得 222232d d (1)3-+=+⎰⎰x y y e x y ，即 2222arctan d 13-+=++⎰x t y y e t C y ， ------6分其中2(0)2arctan (0)1(0)=++f C f f . ------8分若=+∞L ，则对上式取极限→+∞x ，并利用2d 2+∞-=⎰t e t ，得π3=-C .-----10分另一方面，令2()2arctan 1=++ug u u u ，则2223()>0(1)+'=+u g u u ，所以函数()g u 在(, )-∞+∞上严格单调增加. 因此，当(0)1≤f 时，1π((0))(1)2+=≤=C g f g ，但2π1π22+>>C ，矛盾，这就证明了+lim ()→∞=x f x L 为有限数.最后，取max{(0),}=M f L ，则|()|≤f x M ，[0,)∀∈+∞x . -----14分。

滑铁卢数学竞赛

滑铁卢数学竞赛滑铁卢数学竞赛是加拿大滑铁卢大学举办的一项年度数学竞赛活动。

该竞赛旨在通过一系列难度不断增加的数学问题，考察参赛者的数学思维能力、解题能力以及创造力。

每年都有来自世界各地的学生参加该比赛，其中包括来自中小学的学生以及大学生。

滑铁卢数学竞赛分为两个阶段，第一阶段为全球性选拔赛，任何人都可以参加。

参赛者需要在线完成一套由滑铁卢大学编制的数学测试，题型涵盖代数、几何、组合数学等多个数学领域。

根据第一阶段的成绩，滑铁卢大学将选拔出前几百名成绩优异的参赛者晋级到第二阶段。

第二阶段为面试阶段，只有第一阶段晋级的学生才可以参加。

参赛者需要前往滑铁卢大学进行现场的笔试和面试。

笔试部分主要考察参赛者的数学基础知识和解题能力，而面试部分则更加注重参赛者的思维过程和解题思路。

面试时，学生需要与评委进行面对面的交流，展示自己的数学思考能力。

滑铁卢数学竞赛的题目通常非常有难度，涉及到一些高级数学概念和方法。

参赛者需要具备扎实的数学基础知识，并且具备独立思考和解决问题的能力。

竞赛的目的不仅是测试学生的数学水平，更重要的是培养他们解决问题的能力和数学思维方式。

参加滑铁卢数学竞赛对于学生来说是一次宝贵的经历。

这个竞赛可以提供一个展示自己数学才能的平台，也可以锻炼参赛者的思维能力和团队合作精神。

在竞赛中，学生们可以结识来自不同国家和地区的志同道合的数学爱好者，分享彼此的数学体验和解题方法。

滑铁卢数学竞赛也为参赛者提供了一些奖励和机会。

根据参赛者在竞赛中的表现，滑铁卢大学会为他们颁发证书和奖状，并且可以获得一些奖金和奖品。

此外，优秀的参赛者还有机会获得滑铁卢大学的奖学金和入学机会，为他们的未来发展开启了一扇大门。

总之，滑铁卢数学竞赛是一个非常有挑战性和有意义的数学竞赛活动。

通过参加这个竞赛，学生们可以提升自己的数学能力，拓展自己的数学视野，同时也能够展示自己的才能和潜力。

无论是对于中小学生还是大学生，参加滑铁卢数学竞赛都是一个值得鼓励和支持的选择。

Fermat滑铁卢数学竞赛(Grade 11)-数学Mathematics-2002-试题 exam

Canadian Instituteof Actuaries Chartered AccountantsSybasei Anywhere SolutionsScoring:There is no penalty for an incorrect answer.Each unanswered question is worth 2, to a maximum of 10 unanswered questions.Part A: Each correct answer is worth 5.1.If x =3, the numerical value of 522–x is(A ) –1(B ) 27(C ) –13(D )–31(E ) 32.332232++ is equal to(A ) 3(B ) 6(C ) 2(D )32(E ) 53.If it is now 9:04 a.m., in 56 hours the time will be(A ) 9:04 a.m.(B ) 5:04 p.m.(C ) 5:04 a.m.(D ) 1:04 p.m.(E ) 1:04 a.m.4.Which one of the following statements is not true?(A ) 25 is a perfect square.(B ) 31 is a prime number.(C ) 3 is the smallest prime number.(D ) 8 is a perfect cube.(E ) 15 is the product of two prime numbers.5. A rectangular picture of Pierre de Fermat, measuring 20 cmby 40 cm, is positioned as shown on a rectangular postermeasuring 50 cm by 100 cm. What percentage of the areaof the poster is covered by the picture?(A ) 24%(B ) 16%(C ) 20%(D ) 25%(E ) 40%6.Gisa is taller than Henry but shorter than Justina. Ivan is taller than Katie but shorter than Gisa. Thetallest of these five people is(A ) Gisa (B ) Henry (C ) Ivan (D ) Justina (E ) Katie7. A rectangle is divided into four smaller rectangles. Theareas of three of these rectangles are 6, 15 and 25, as shown.The area of the shaded rectangle is(A ) 7(B ) 15(C ) 12(D ) 16(E) 108.In the diagram, ABCD and DEFG are squares with equal side lengths, and ∠=°DCE 70. The value of y is (A ) 120(B ) 160(C ) 130(D ) 110(E ) 1409.The numbers 1 through 20 are written on twenty golf balls, with one number on each ball. The golfballs are placed in a box, and one ball is drawn at random. If each ball is equally likely to be drawn,what is the probability that the number on the golf ball drawn is a multiple of 3?(A )320(B )620(C )1020(D )520(E )12010.ABCD is a square with AB x =+16 and BC x =3, as shown.The perimeter of ABCD is(A ) 16(B ) 32(C ) 96(D ) 48(E ) 24Part B: Each correct answer is worth 6.11. A line passing through the points 02,−() and 10,() also passes through the point 7,b (). The numericalvalue of b is(A ) 12(B )92(C ) 10(D ) 5(E ) 1412.How many three-digit positive integers are perfect squares?(A ) 23(B ) 22(C ) 21(D ) 20(E ) 1913. A “double-single” number is a three-digit number made up of two identical digits followed by adifferent digit. For example, 553 is a double-single number. How many double-single numbers are there between 100 and 1000?(A ) 81(B ) 18(C ) 72(D ) 64(E ) 9014.The natural numbers from 1 to 2100 are entered sequentially in 7 columns, with the first 3 rows asshown. The number 2002 occurs in column m and row n . The value of m n + isColumn 1Column 2Column 3Column 4Column 5Column 6Column 7Row 1 1 2 3 4 5 6 7Row 2 8 91011121314Row 315161718192021M M M M M M M M(A ) 290(B ) 291(C ) 292(D ) 293(E ) 294x + 163xA BD C15.In a sequence of positive numbers, each term after the first two terms is the sum of all of the previousterms . If the first term is a ,the second term is 2, and the sixth term is 56, then the value of a is(A ) 1(B ) 2(C ) 3(D ) 4(E ) 516.If ac ad bc bd +++=68 and c d +=4, what is the value of a b c d +++?(A ) 17(B ) 85(C ) 4(D ) 21(E ) 6417.The average age of a group of 140 people is 24. If the average age of the males in the group is 21 andthe average age of the females is 28, how many females are in the group?(A ) 90(B ) 80(C ) 70(D ) 60(E ) 5018. A rectangular piece of paper AECD has dimensions 8 cm by 11 cm. Corner E is folded onto point F , which lies on DC ,as shown. The perimeter of trapezoid ABCD is closest to (A ) 33.3 cm (B ) 30.3 cm (C ) 30.0 cm(D ) 41.3 cm (E ) 35.6 cm 19.If 238610a b =(), where a and b are integers, then b a − equals(A ) 0(B ) 23(C )−13(D )−7(E )−320.In the diagram, YQZC is a rectangle with YC =8 and CZ = 15. Equilateral triangles ABC and PQR , each withside length 9, are positioned as shown with R and B on sidesYQ and CZ , respectively. The length of AP is (A ) 10(B )117(C ) 9(D ) 8(E )72Part C: Each correct answer is worth 8.21.If 31537521219⋅⋅⋅⋅+−=L n n , then the value of n is(A ) 38(B ) 1(C ) 40(D ) 4(E ) 3922.The function f x () has the property that f x y f x f y xy +()=()+()+2, for all positive integers x and y .If f 14()=, then the numerical value of f 8() is(A ) 72(B ) 84(C ) 88(D ) 64(E ) 80continued ...Figure 1Figure 223.The integers from 1 to 9 are listed on a blackboard. If an additional m eights and k nines are added tothe list, the average of all of the numbers in the list is 7.3. The value of k m + is(A ) 24(B ) 21(C ) 11(D ) 31(E ) 8924. A student has two open-topped cylindrical containers. (Thewalls of the two containers are thin enough so that theirwidth can be ignored.) The larger container has a height of20 cm, a radius of 6 cm and contains water to a depth of 17cm. The smaller container has a height of 18 cm, a radius of5 cm and is empty. The student slowly lowers the smallercontainer into the larger container, as shown in the cross-section of the cylinders in Figure 1. As the smaller container is lowered, the water first overflows out of the larger container (Figure 2) and then eventually pours into thesmaller container. When the smaller container is resting onthe bottom of the larger container, the depth of the water in the smaller container will be closest to(A ) 2.82 cm (B ) 2.84 cm (C ) 2.86 cm(D ) 2.88 cm (E ) 2.90 cm25.The lengths of all six edges of a tetrahedron are integers. The lengths of five of the edges are 14, 20,40, 52, and 70. The number of possible lengths for the sixth edge is(A ) 9(B ) 3(C ) 4(D ) 5(E ) 6。

加拿大数学竞赛历年试题(滑铁卢大学)

Each question is worth 10 marks
Calculating devices are allowed, provided that they do not have any of the following features: (i) internet access, (ii) the ability to communicate with other devices, (iii) information previously stored by students (such as formulas, programs, notes, etc.), (iv) a computer algebra system, (v) dynamic geometry software.
Parts of each question can be of two types: 1. SHORT ANSWER parts indicated by
• worth 3 marks each • full marks given for a correct answer which is placed in the box • part marks awarded only if relevant work is shown in the space provided
WRITE ALL ANSWERS IN THE ANSWER BOOKLET PROVIDED. • Extra paper for your ﬁnished solutions must be supplied by your supervising teacher and inserted into your answer booklet. Write your name, school name, and question number on any inserted pages. • Express answers as simpli√ﬁed exact numbers except where otherwise indicated. For example, π + 1 and 1 − 2 are simpliﬁed exact numbers.

Cayley滑铁卢数学竞赛(Grade 10)-数学Mathematics-1998-试题 exam

Chartered Accountants SybaseInc. (Waterloo) IBMCanada Ltd.Canadian Institute of ActuariesDo not open the contest booklet until you are told to do so.You may use rulers, compasses and paper for rough work.Calculators are permitted, providing they are non-programmable and without graphic displays.Part A: Each question is worth 5 credits.1.The value of 03012..()+ is(A ) 0.7(B ) 1(C ) 0.1(D ) 0.19(E ) 0.1092.The pie chart shows a percentage breakdown of 1000 votesin a student election. How many votes did Sue receive?(A ) 550(B ) 350(C ) 330(D ) 450(E ) 9353.The expression a a a 9153× is equal to(A ) a 45(B ) a 8(C ) a 18(D ) a 14(E ) a 214.The product of two positive integers p and q is 100. What is the largest possible value of p q +?(A ) 52(B ) 101(C ) 20(D ) 29(E ) 255.In the diagram, ABCD is a rectangle with DC =12. If the area of triangle BDC is 30, what is the perimeter ofrectangle ABCD ?(A ) 34(B ) 44(C ) 30(D ) 29(E ) 606.If x =2 is a solution of the equation qx –311=, the value of q is (A ) 4(B ) 7(C ) 14(D ) –7(E ) –47.In the diagram, AB is parallel to CD . What is the value ofy ?(A ) 75(B ) 40(C ) 35(D ) 55(E ) 508.The vertices of a triangle have coordinates 11,(), 71,() and 53,(). What is the area of this triangle?(A ) 12(B ) 8(C ) 6(D ) 7(E ) 99.The number in an unshaded square is obtained by adding thenumbers connected to it from the row above. (The ‘11’ is one such number.) The value of x must be (A ) 4(B ) 6(C ) 9(D ) 15(E) 10Scoring:There is no penalty for an incorrect answer.Each unanswered question is worth 2 credits, to a maximum of 20 credits.A BCD DAC B10.The sum of the digits of a five-digit positive integer is 2. (A five-digit integer cannot start with zero.)The number of such integers is(A ) 1(B ) 2(C ) 3(D ) 4(E ) 5Part B: Each question is worth 6 credits.11.If x y z ++=25, x y +=19 and y z +=18, then y equals(A ) 13(B ) 17(C ) 12(D ) 6(E ) –612. A regular pentagon with centre C is shown. The value of xis(A ) 144(B ) 150(C ) 120(D ) 108(E ) 7213.If the surface area of a cube is 54, what is its volume?(A ) 36(B ) 9(C ) 8138(D ) 27(E ) 162614.The number of solutions x y ,() of the equation 3100x y +=, where x and y are positive integers, is(A ) 33(B ) 35(C ) 100(D ) 101(E ) 9715.If y –55= and 28x =, then x y + equals(A ) 13(B ) 28(C ) 3316.Rectangle ABCDhas length 9 and width 5. Diagonal is divided into 5 equal parts at W , X , Y , and Z area of the shaded region.(A ) 36(B ) 365(C ) 18(D ) 41065(E ) 2106517.If N p q =()()()+75243 is a perfect cube, where p and q are positive integers, the smallest possible valueof p q + is(A ) 5(B ) 2(C ) 8(D ) 6(E ) 1218.Q is the point of intersection of the diagonals of one face ofa cube whose edges have length 2 units. The length of QRis(A ) 2(B ) 8(C ) 5(D ) 12(E ) 619.Mr. Anderson has more than 25 students in his class. He has more than 2 but fewer than 10 boys andmore than 14 but fewer than 23 girls in his class. How many different class sizes would satisfy these conditions?(A ) 5(B ) 6(C ) 7(D ) 3(E ) 420.Each side of square ABCD is 8. A circle is drawn through A and D so that it is tangent to BC . What is the radius of thiscircle?(A ) 4(B ) 5(C ) 6(D ) 42(E ) 5.25Part C: Each question is worth 8 credits.21.When Betty substitutes x =1 into the expression ax x c 32–+ its value is –5. When she substitutesx =4 the expression has value 52. One value of x that makes the expression equal to zero is(A ) 2(B ) 52(C ) 3(D ) 72(E ) 422. A wheel of radius 8 rolls along the diameter of a semicircleof radius 25 until it bumps into this semicircle. What is thelength of the portion of the diameter that cannot be touchedby the wheel?(A ) 8(B ) 12(C ) 15(D ) 17(E ) 2023.There are four unequal, positive integers a , b , c , and N such that N a b c =++535. It is also true thatN a b c =++454 and N is between 131 and 150. What is the value of a b c ++?(A ) 13(B ) 17(C ) 22(D ) 33(E ) 3624.Three rugs have a combined area of 2002m . By overlapping the rugs to cover a floor area of 1402m ,the area which is covered by exactly two layers of rug is 242m . What area of floor is covered by three layers of rug?(A ) 122m (B ) 182m (C ) 242m (D) 362m (E ) 422m 25.One way to pack a 100 by 100 square with 10000 circles, each of diameter 1, is to put them in 100rows with 100 circles in each row. If the circles are repacked so that the centres of any three tangent circles form an equilateral triangle, what is the maximum number of additional circles that can be packed?(A ) 647(B ) 1442(C ) 1343(D) 1443(E ) 1344。

2009PascalSolution滑铁卢竞赛题答案

1.Calculating,2×9−√36+1=18−6+1=13.Answer:(D)2.On Saturday,Deepit worked6hours.On Sunday,he worked4hours.Therefore,he worked6+4=10hours in total on Saturday and Sunday.Answer:(E) 3.Since1piece of gum costs1cent,then1000pieces of gum cost1000cents.Since there are100cents in a dollar,the total cost is$10.00.Answer:(D) 4.Since each of the18classes has28students,then there are18×28=504students who attendthe school.On Monday,there were496students present,so504−496=8students were absent.Answer:(A) 5.The sum of the angles around any point is360◦.Therefore,5x◦+4x◦+x◦+2x◦=360◦or12x=360or x=30.Answer:(D) 6.When−1is raised to an even exponent,the result is1.When−1is raised to an odd exponent,the result is−1.Thus,(−1)5−(−1)4=−1−1=−2.Answer:(A) 7.Since P Q is horizontal and the y-coordinate of P is1,then the y-coordinate of Q is1.Since QR is vertical and the x-coordinate of R is5,then the x-coordinate of Q is5.Therefore,the coordinates of Q are(5,1).Answer:(C)8.When y=3,we have y3+yy2−y=33+332−3=27+39−3=306=5.Answer:(D)9.Since there are4♣’s in each of theﬁrst two columns,then at least1♣must be moved out ofeach of these columns to make sure that each column contains exactly three♣’s.Therefore,we need to move at least2♣’s in total.If we move the♣from the top left corner to the bottom right corner♣♣♣♣♣♣♣♣♣♣♣♣♣♣♣and the♣from the second row,second column to the third row,ﬁfth column♣♣♣♣♣♣♣♣♣♣♣♣♣♣♣then we have exactly three♣’s in each row and each column.Therefore,since we must move at least2♣’s and we can achieve the conﬁguration that we want by moving2♣’s,then2is the smallest number.(There are also other combinations of moves that will give the required result.)Answer:(B) 10.Solution1Since z=4and x+y=7,then x+y+z=(x+y)+z=7+4=11.Solution2Since z=4and x+z=8,then x+4=8or x=4.Since x=4and x+y=7,then4+y=7or y=3.Therefore,x+y+z=4+3+4=11.Answer:(C) 11.We write out theﬁve numbers to5decimal places each,without doing any rounding:5.076=5.07666...5.076=5.07676...5.07=5.070005.076=5.076005.076=5.07607...We can use these representations to order the numbers as5.07000,5.07600,5.07607...,5.07666...,5.07676...so the number in the middle is5.076.Answer:(E) 12.Solution1Since there are24hours in a day,Francis spends13×24=8hours sleeping.Also,he spends14×24=6hours studying,and18×24=3hours eating.The number of hours that he has left is24−8−6−3=7hours. Solution2Francis spends13+14+18=8+6+324=1724of a day either sleeping,studying or eating.This leaves him1−1724=724of his day.Since there are24hours in a full day,then he has7hours left.Answer:(D) 13.Solution1Since the sum of the angles in a triangle is180◦,then∠QP S=180◦−∠P QS−∠P SQ=180◦−48◦−38◦=94◦Therefore,∠RP S=∠QP S−∠QP R=94◦−67◦=27◦.Solution2Since the sum of the angles in a triangle is180◦,then∠QRP=180◦−∠P QR−∠QP R=180◦−48◦−67◦=65◦Therefore,∠P RS=180◦−∠P RQ=180◦−65◦=115◦.Using P RS,∠RP S=180◦−∠P RS−∠P SR=180◦−115◦−38◦=27◦Answer:(A)14.The perimeter of the shaded region equals the sum of the lengths of OP and OQ plus the lengthof arc P Q.Each of OP and OQ has length5.Arc P Q forms34of the circle with centre O and radius5,because the missing portion corre-sponds to a central angle of90◦,and so is14of the total circle.Thus,the length of arc P Q is34of the circumference of this circle,or34(2π(5))=152π.Therefore,the perimeter is5+5+152π≈33.56which,of the given answers,is closest to34.Answer:(A)15.After some trial and error,we obtain the two lists{4,5,7,8,9}and{3,6,7,8,9}.Why are these the only two?If the largest number of theﬁve integers was8,then the largest that the sum could be would be8+7+6+5+4=30,which is too small.This tells us that we must include one9in the list.(We cannot include any number larger than9,since each number must be a single-digit number.)Therefore,the sum of the remaining four numbers is33−9=24.If the largest of the four remaining numbers is7,then their largest possible sum would be 7+6+5+4=22,which is too small.Therefore,we also need to include an8in the list.Thus,the sum of the remaining three numbers is24−8=16.If the largest of the three remaining numbers is6,then their largest possible sum would be 6+5+4=15,which is too small.Therefore,we also need to include an7in the list.Thus,the sum of the remaining two numbers is16−7=9.This tells us that we need two diﬀerent positive integers,each less than7,that add to9.These must be3and6or4and5.This gives us the two lists above,and shows that they are the only two such lists.Answer:(B) 16.The area of the entire grid is4×9=36.The area of P QR is12(QR)(P Q)=12(3)(4)=6.The area of ST U is12(ST)(UT)=12(4)(3)=6.The area of the rectangle with base RS is2×4=8.Therefore,the total shaded area is6+6+8=20and so the unshaded area is36−20=16.The ratio of the shaded area to the unshaded area is20:16=5:4.Answer:(E) 17.We can suppose that each test is worth100marks.Since the average of herﬁve test marks is73%,then the total number of marks that she received is5×73=365.Once her teacher removes a mark,her new average is76%so the sum of the remaining four marks is4×76=304.Since365−304=61,then the mark removed was61%.Answer:(B) 18.Solution1From December31,1988to December31,2008,a total of20years have elapsed.A time period of20years is the same asﬁve4year periods.Thus,the population of Arloe has doubled 5times over this period to its total of 3456.Doubling 5times is equivalent to multiplying by 25=32.Therefore,the population of Arloe on December 31,1988was 345632=108.Solution 2The population doubles every 4years going forward,so is halved every 4years going backwards in time.The population on December 31,2008was 3456.The population on December 31,2004was 3456÷2=1728.The population on December 31,2000was 1728÷2=864.The population on December 31,1996was 864÷2=432.The population on December 31,1992was 432÷2=216.The population on December 31,1988was 216÷2=108.Answer:(D)19.Since Pat drives 60km at 80km/h,this takes him 60km 80km/h =34h.Since Pat has 2hours in total to complete the trip,then he has 2−34=54hours left to complete the remaining 150−60=90km.Therefore,he must travel at 90km 54h =360km/h =72km/h.Answer:(C)20.Since the three numbers in each straight line must have a product of 3240and must include 45,then the other two numbers in each line must have a product of 3240=72.The possible pairs of positive integers are 1and 72,2and 36,3and 24,4and 18,6and 12,and 8and 9.The sums of the numbers in these pairs are 73,38,27,22,18,and 17.To maximize the sum of the eight numbers,we want to choose the pairs with the largest possible sums,so we choose the ﬁrst four pairs.Thus,the largest possible sum of the eight numbers is 73+38+27+22=160.Answer:(E)21.Since each of Alice and Bob rolls one 6-sided die,then there are 6×6=36possible combinationsof rolls.Each of these 36possibilities is equally likely.Alice wins when the two values rolled diﬀer by 1.The possible combinations that diﬀer by 1are (1,2),(2,3),(3,4),(4,5),(5,6),(2,1),(3,2),(4,3),(5,4),and (6,5).Therefore,there are 10combinations when Alice wins.Thus her probability of winning is 1036=518.Answer:(C)22.Diameters P Q and RS cross at the centre of the circle,which we call O .The area of the shaded region is the sum of the areas of P OS and ROQ plus the sum of the areas of sectors P OR and SOQ .Each of P OS and ROQ is right-angled and has its two perpendicular sides of length 4(the radius of the circle).Therefore,the area of each of these triangles is 12(4)(4)=8.Each of sector P OR and sector SOQ has area 14of the total area of the circle,as each hascentral angle 90◦(that is,∠P OR =∠SOQ =90◦)and 90◦is one-quarter of the total central angle.Therefore,each sector has area 14(π(42))=14(16π)=4π.Thus,the total shaded area is 2(8)+2(4π)=16+8π.Answer:(E)23.The maximum possible mass of a given coin is 7×(1+0.0214)=7×1.0214=7.1498g.The minimum possible mass of a given coin is 7×(1−0.0214)=7×0.9786=6.8502g.What are the possible numbers of coins that could make up 1000g?To ﬁnd the largest number of coins,we want the coins to be as light as possible.If all of the coins were as light as possible,we would have 10006.8502≈145.98coins.Now,we cannot have a non-integer number of coins.This means that we must have at most 145coins.(If we had 146coins,the total mass would have to be at least 146×6.8502=1000.1292g,which is too heavy.)Practically,we can get 145coins to have a total mass of 1000g by taking 145coins at the minimum possible mass and making each slightly heavier.To ﬁnd the smallest number of coins,we want the coins to be as heavy as possible.If all of the coins were as heavy as possible,we would have 10007.1498≈139.86coins.Again,we cannot have a non-integer number of coins.This means that we must have at least 140coins.(If we had 139coins,the total mass would be at most 139×7.1498=993.8222g,which is too light.)Therefore,the diﬀerence between the largest possible number and smallest possible number of coins is 145−140=5.Answer:(B)24.Divide the large cube of side length 40into 8smaller cubes of side length 20,by making threecuts of the large cube through its centre using planes parallel to the pairs of faces.Each of these small cubes has the centre of the large cube as its vertex.Each of these small cubes also just encloses one of the large spheres,in the sense that the sphere just touches each of the faces of the small cube.We call the sphere that ﬁts in the central space the inner sphere.To make this sphere as large possible,its centre will be at the centre of the large cube.(If this was not the case,the centre be outside one of the small cubes,and so would be farther away from one of the large spheres than from another.)To ﬁnd the radius of the inner sphere,we must ﬁnd the shortest distance from the centre of the large cube (that is,the centre of the inner sphere)to one of the large spheres.(Think of starting the inner sphere as a point at the centre of the cube and inﬂating it until it just touches the large spheres.)Consider one of these small cubes and the sphere inside it.Join the centre of the small cube to one of its vertices.Since the small cube has side length 20,then this new segment has length √102+102+102or √300,since to get from the centre to a vertex,we must go over 10,down 10and across 10.(See below for an explanation of why this distance is thus √300.)The inner sphere will touch the large sphere along this segment.Thus,the radius of the inner sphere will be this distance (√300)minus the radius of the large sphere (10),and so is √300−10≈7.32.Of the given answers,this is closest to 7.3.(We need to justify why the distance from the centre of the small cube to its vertex is √102+102+102.Divide the small cube into 8tiny cubes of side length 10each.The distance from the centre of the small cube to its vertex is equal to the length of a diagonal of one of the tiny cubes.Consider a rectangular prism with edge lengths a ,b and c .What is the length,d ,of the diag-onal inside the prism?By the Pythagorean Theorem,a face with side lengths a and b has a diagonal of length √a 2+b 2.Consider the triangle formed by this diagonal,the diagonal of the prism and one of the vertical edges of the prism,of length c .cThis triangle is right-angled since the vertical edge is perpendicular to the top face.By the Pythagorean Theorem again,d 2=(√a 2+b 2)2+c 2,so d 2=a 2+b 2+c 2or d =√a 2+b 2+c 2.)Answer:(B)25.The three machines operate in a way such that if the two numbers in the output have a commonfactor larger than 1,then the two numbers in the input would have to have a common factor larger than 1.To see this,let us look at each machine separately.We use the fact that if two numbers are each multiples of d ,then their sum and diﬀerence are also multiples of d .Suppose that (m,n )is input into Machine A.The output is (n,m ).If n and m have a common factor larger than 1,then m and n do as well.Suppose that (m,n )is input into Machine B.The output is (m +3n,n ).If m +3n and n have a common factor d ,then (m +3n )−n −n −n =m has a factor of d as each part of the subtraction is a multiple of d .Therefore,m and n have a common factor of d .Suppose that (m,n )is input into Machine C.The output is (m −2n,n ).If m −2n and n have a common factor d ,then (m −2n )+n +n =m has a factor of d as each part of the addition is a multiple of d .Therefore,m and n have a common factor of d .In each case,any common factor that exists in the output is present in the input.Let us look at the numbers in the ﬁve candidates.After some work,we can ﬁnd the prime factorizations of the six integers:2009=7(287)=7(7)(41)1016=8(127)=2(2)(2)(127)1004=4(251)=2(2)(251)1002=2(501)=2(3)(167)1008=8(126)=8(3)(42)=16(3)(3)(7)=2(2)(2)(2)(3)(3)(7)1032=8(129)=8(3)(43)=2(2)(2)(3)(43)Therefore,the only one of 1002,1004,1008,1016,1032that has a common factor larger than 1with 2009is 1008,which has a common factor of 7with 2009.How does this help?Since2009and1008have a common factor of7,then whatever pair was input to produce(2009,1008)must have also had a common factor of7.Also,the pair that was input to create this pair also had a common factor of7.This can be traced back through every step to say that the initial pair that produces the eventual output of(2009,1008)must have a common factor of7.Thus,(2009,1008)cannot have come from(0,1).Notes:•This does not tell us that the other four pairs necessarily work.It does tell us,though, that(2009,1008)cannot work.•We can trace the other four outputs back to(0,1)with some eﬀort.(This process is easier to do than it is to describe!)To do this,we notice that if the output of Machine A was(a,b),then its input was(b,a), since Machine A switches the two entries.Also,if the output of Machine B was(a,b),then its input was(a−3b,b),since MachineB adds three times the second number to theﬁrst.Lastly,if the output of Machine C was(a,b),then its input was(a+2b,b),since MachineC subtracts two times the second number from theﬁrst.Consider(2009,1016)for example.We try toﬁnd a way from(2009,1016)back to(0,1).We only need toﬁnd one way that works,rather than looking for a speciﬁc way.We note before doing this that starting with an input of(m,n)and then applying MachineB then MachineC gives an output of((m+3n)−2n,n)=(m+n,n).Thus,if applyingMachine B then Machine C(we call this combination“Machine BC”)gives an output of (a,b),then its input must have been(a−b,b).We can use this combined machine to try to work backwards and arrive at(0,1).This will simplify the process and help us avoid negative numbers.We do this by making a chart and by attempting to make the larger number smaller wher-ever possible:Output Machine Input(2009,1016)BC(993,1016)(993,1016)A(1016,993)(1016,993)BC(23,993)(23,993)A(993,23)(993,23)BC,43times(4,23)(4,23)A(23,4)(23,4)BC,5times(3,4)(3,4)A(4,3)(4,3)BC(1,3)(1,3)A(3,1)(3,1)B(0,1)Therefore,by going up through this table,we can see a way to get from an initial input of(0,1)to aﬁnal output of(2009,1016).In a similar way,we can show that we can obtainﬁnal outputs of each of(2009,1004), (2009,1002),and(2009,1032).Answer:(D)。

2012滑铁卢竞赛试题答案

4 12
=1 . 3
(b) Since the two lines are parallel, then their slopes are equal. We re-write the given equations in the form “y = mx + b”. 2 The ﬁrst equation becomes 6y = −4x + 5 or y = − 4 x+ 5 or y = − 3 x+ 5 . 6 6 6 Since the ﬁrst line is not vertical, then the second line is not vertical, and so k = 0. 3 6 x+ k . The second equation becomes ky = −6x + 3 or y = − k 2 6 k 3 3 Therefore, − 3 = − k and so 6 = 2 or k = 6 × 2 = 9. (c) Adding the two equations, we obtain x + x2 = 2 or x2 + x − 2 = 0. Factoring, we obtain (x + 2)(x − 1) = 0, and so x = −2 or x = 1. From the ﬁrst equation, y = −x. If x = −2, then y = 2 and if x = 1, then y = −1. Therefore, the solutions are (x, y ) = (−2, 2) and (x, y ) = (1, −1). (We can check that each of these solutions satisﬁes both equations.) of the mass (or 50 g) is salt and the 3. (a) Since the 200 g solution is 25% salt by mass, then 1 4 rest (150 g) is water. When water is added, the mass of salt does not change. Therefore, the 50 g of salt initially 1 in the solution becomes 10% (or 10 ) of the ﬁnal solution by mass. Therefore, the total mass of the ﬁnal solution is 10 × 50 = 500 g. Thus, the mass of water added is 500 − 200 = 300 g.

2014CIMC滑铁卢国际加拿大数学竞赛

Canadian Intermediate Mathematics Contest NOTE:1.Please read the instructions on the front cover of this booklet.2.Write solutions in the answer booklet provided.3.It is expected that all calculations and answers will be expressed as exact numbers such as 4π,2+√7,etc.,rather than as 12.566...or4.646....4.While calculators may be used for numerical calculations,other mathematical steps must be shown and justiﬁed in your written solutions and speciﬁc marks may be allocated for these steps.For example,while your calculator might be able to ﬁnd the x -intercepts of the graph of an equation like y =x 3−x ,you should show the algebraic steps that you used to ﬁnd these numbers,rather than simply writing these numbers down.5.Diagrams are not drawn to scale.They are intended as aids only.6.No student may write both the Canadian Senior Mathematics Contest and the Canadian Intermediate Mathematics Contest in the same year.PART AFor each question in Part A,full marks will be given for a correct answer which is placed in the box.Part marks will be awarded only if relevant work is shown in the space provided in the answer booklet.1.There are 200people at the beach,and 65%of these people are children.If 40%of the children are swimming,how many children are swimming?2.If x +2y =14and y =3,what is the value of 2x +3y ?3.In the diagram,ABCD is a rectangle with points Pand Q on AD so that AB =AP =P Q =QD .Also,point R is on DC with DR =RC .If BC =24,whatis the area of P QR ?A B CD P Q R 4.At a given time,the depth of snow in Kingston is 12.1cm and the depth of snow in Hamilton is 18.6cm.Over the next thirteen hours,it snows at a constant rate of2.6cm per hour in Kingston and at a constant rate of x cm per hour in Hamilton.At the end of these thirteen hours,the depth of snow in Kingston is the same as the depth of snow in Hamilton.What is the value of x ?5.Scott stacks golfballs to make a pyramid.The ﬁrst layer,or base,of the pyramid is a square of golfballs and rests on a ﬂat table.Each golfball,above the ﬁrst layer,rests in a pocket formed by four golfballs in the layer below (as shown in Figure 1).Each layer,including the ﬁrst layer,is completely ﬁlled.For example,golfballs can be stacked into a pyramid with 3levels,as shown in Figure 2.The four triangular faces of the pyramid in Figure 2include a total of exactly 13diﬀerent golfballs.Scott makes a pyramid in which the four triangular faces include a total of exactly 145diﬀerent golfballs.How many layers does this pyramid have?Figure 1Figure 26.A positive integer is a prime number if it is greater than1and has no positive divisorsother than1and itself.For example,the number5is a prime number because its only two positive divisors are1and5.The integer43797satisﬁes the following conditions:•each pair of neighbouring digits(read from left to right)forms a two-digit primenumber,and•all of the prime numbers formed by these pairs are diﬀerent,because43,37,79,and97are all diﬀerent prime numbers.There are many integers with more thanﬁve digits that satisfy both of these conditions.What is the largest positive integer that satisﬁes both of these conditions?PART BFor each question in Part B,your solution must be well organized and contain words of explanation or justiﬁcation.Marks are awarded for completeness,clarity,and style of presentation.A correct solution,poorly presented,will not earn full marks.1.(a)Determine the average of the six integers22,23,23,25,26,31.(b)The average of the three numbers y+7,2y−9,8y+6is27.What is the valueof y?(c)Four positive integers,not necessarily diﬀerent and each less than100,have anaverage of94.Determine,with explanation,the minimum possible value forone of these integers.2.(a)In the diagram, P QR is right-angled at R.If P Q=25and RQ=24,determine the perimeterand area of P QR.PQR(b)In the diagram, ABC is right-angled at C withAB=c,AC=b,and BC=a.Also, ABC has perimeter144and area504.Determine all possible values of c.(You may use the facts that,for any numbers x and y, (x+y)2=x2+2xy+y2and(x−y)2=x2−2xy+y2.)AB C ab cCanadian Intermediate Mathematics Contest(English)20143.Vicky starts with a list(a,b,c,d)of four digits.Each digit is0,1,2,or3.Vickyenters the list into a machine to produce a new list(w,x,y,z).In the new list,w is the number of0s in the original list,while x,y and z are the numbers of1s,2s and3s,respectively,in the original list.For example,if Vicky enters(1,3,0,1),the machine produces(1,2,0,1).(a)What does the machine produce when Vicky enters(2,3,3,0)?(b)Vicky enters(a,b,c,d)and the machine produces the identical list(a,b,c,d).Determine all possible values of b+2c+3d.(c)Determine all possible lists(a,b,c,d)with the property that when Vicky enters(a,b,c,d),the machine produces the identical list(a,b,c,d).(d)Vicky buys a new machine into which she can enter a list of ten digits.Eachdigit is0,1,2,3,4,5,6,7,8,or9.The machine produces a new list whoseentries are,in order,the numbers of0s,1s,2s,3s,4s,5s,6s,7s,8s,and9s inthe original list.Determine all possible lists,L,of ten digits with the propertythat when Vicky enters L,the machine produces the identical list L.。

历届国际数学竞赛试题

历届国际数学竞赛试题历届国际数学竞赛试题求证(21n 4)/(14n 3) 对每个自然数n都是最简分数。

2.√2；(b)A=1；(c)A=2。

3. a试作一直角三角形使其斜边为已知的c，斜边上的中线是两直角边的几何平均值。

5.、MBEF，这两个正方形的外接圆的圆心分别是P、Q，设这两个外接圆又交于M、N，(a.)当M在A与B之间变动时，求线断PQ的中点的轨迹。

6.找出所有具有下列性质的三位数N：N能被11整除且N/11等于N的各位数字的平方和。

2.√(12x))23.已知从A、B引出的高线长度以及从A引出的中线长，求作三角形ABC。

5.求XY中点的轨迹；b.一个圆锥内有一内接球，又有一圆柱体外切于此圆球，其底面落在圆锥的底面上。

令V1 为圆锥的体积，V2 为圆柱的体积。

(a). 求证：V1不等于V2 ；(b). 求V1/V2的最小值；并在此情况下作出圆锥顶角的一般。

7.设a、b是常数，解方程组x y z =a; x2 y2 z2 =b2; xy=z2并求出若使x、y、z是互不相同的正数，a、b应满足什么条件？2.解方程cosx - sinx = 1, 其中n是一个自然数。

4. P是三角形ABC内部一点，PA交BC于D，PB交AC 于E，PC交AB于F，求证AP/PD, BP/PE, CP/PF 中至少有一个不大于2，也至少有一个不小于2。

5三个不共线的点A、B、C，平面p不平行于ABC，并且A、B、C在p的同一侧。

在p上任意取三个点A', B', C'，A'', B'', C''设分别是边AA', BB', CC'的中点，O是三角形A''B''C''的重心。

问，当A',B',C'变化时，O的轨迹是什么？nn第4届IMO1.试找出满足下列不等式的所有实数x：√(3-x)- √(x 1) > 1/2.3.解方程cosxcos2x cos3x = 1。

滑铁卢数学竞赛

滑铁卢数学竞赛年1月10日更新这篇主要来介绍一下滑铁卢系列数学竞赛，下面是该项赛事的特点以及备考建议：【特点】（1）全年龄段，从7年级到12年级都有，建议参加12年级Euclid 竞赛；（2）全球统考，高含金量，对于申请有帮助；（2）有些比赛项目并不是选择题，是填空、简答题，对于英语表达有一定要求；（3）难度相对比较小，获个奖比较容易；【建议】做真题！做真题！做真题！真题网址：下面是Waterloo数学竞赛的详细介绍以及21年Euclid真题卷：滑铁卢大学始建于1957年，在加拿大最权威的教育杂志Maclean's （麦克林）的排名榜上，连续五年综合排名第一第二。

滑铁卢大学设有加拿大唯一一所数学学院，这也是北美乃至全世界最大的数学学院，因滑铁卢大学在数学领域的优良声誉及传统，以及欧几里德数学竞赛考察标准的严格性和专业性，该竞赛成绩在加拿大和美国大学中已经得到广泛认可，被誉为类似加拿大“数学托福”的考试。

官方网址：【比赛特点】（1）可选择的比赛种类非常多，有适合各种不同年龄段学生的比赛；（2）比赛难度相对较小，比较适合想参加数学类竞赛，但本身数学程度并不是特别出挑的学生；（3）在加拿大的高校中有比较大的影响力，尤其是Euclid的比赛成绩；（4）部分赛题以测试学生的分析能力、逻辑思维能力为主，在2021年MAT考试中出现了21年Euclid的类似试题。

【比赛形式】注：上述数学竞赛都可以使用计算器。

【2021-2022赛程】大家可以看到Waterloo数学竞赛各项赛事跨度比较大，主要集中在四、五月份，特别是Euclid数学竞赛，是一项含金量比较高的全球数学竞赛。

【报名方式】当地可提供报名的机构或以自己学校的名义注册报名注：Waterloo报名时需要填写一个学校代码，所以一般需要通过学校报名。

【奖项设置】（1）国际生全球排名前25%的学生将获得杰出荣誉证书，2021年大概68分；（2）在参赛学校中成绩最高的学生会得到一个校级冠军的奖牌；（3）每位参赛者都可以获得一个参赛证书；从上述奖项的设置可以看出，我们参加Waterloo数学竞赛获一个奖相对而言是比较容易的。

滑铁卢大学数学竞赛

加拿大滑铁卢大学举办费马国际数学竞赛及欧几里得数学竞赛。

滑铁卢大学是加拿大综合排名第三的大学，其创新精神在加拿大排名第一，该大学面向青少年举办的数学竞赛，在全球具有影响力。

费马国际数学竞赛面向高一学生的费马数学竞赛，考试时间60为分钟，满分为150分，其间学生必须面对全英语试卷解答问题。

欧几里得数学竞赛据360教育集团介绍，面向高二学生的欧几里得数学竞赛，考试时间150为分钟，满分为100分，其间学生必须面对全英语试卷解答问题。

最初的数学考试是由安大略省西南部的几个高中老师联合创办的，从六十年代初年每年300人参加考试到今天，累计已经有21万名学生参加了这个考试。

根据滑铁卢大学的校方统计资料：21万名学生中有40%是来自安大略省的学生，20%是来自英属哥伦比亚省的学生，35%是来自加拿大其他省份的，还有5%是来自国际学生，包括美国、英国、中国等世界各国的学生。

2003年因为安大略省取消13年级，部分涉及微积分的试题不再使用，于是将迪卡尔（法国著名数学家）数学竞赛（DescartesContest）更名为（欧几里德数学竞赛）。

现在，欧几里德数学竞赛的分数已经成为Waterloo数学学院各专业以及“软件工程”专业入学录取的重要指标，更成为学生申请该学院奖学金的重要考核标准。

欧几里德数学竞赛（EuclidContest）主要是为高二年级（加拿大11年级）的高中学生提供的考试，考试内容主要包括：代数（函数、三角、排列、组合）、平面组合、解析几何等，他不仅仅看的是结果，更看重的是学生的解题思路和技巧。

考试的及格分数每年大概在40分左右。

因滑铁卢大学在数学领域的优良声誉及传统，以及欧几里德数学竞赛考察标准的严格性和专业性，该竞赛成绩在加拿大大学中已经得到广泛认可，被誉为类似加拿大“数学托福”的考试。

滑铁卢大学数学竞赛。

加拿大欧几里得数学竞赛真题解析2014EuclidSolution

1.(a)Evaluating,√16+√9√16+9=4+3√25=75.(b)Since the sum of the angles in a triangle is 180◦,then (x −10)◦+(x +10)◦+x ◦=180◦or (x −10)+(x +10)+x =180.Thus,3x =180and so x =60.(c)Suppose Bart earns $x per hour.In 4hours,he earns 4×$x =$4x .Then Lisa earns $2x per hour.In 6hours,she earns 6×$2x =$12x .Since they earn $200in total,then 4x +12x =200or 16x =200.Therefore,x =12.5.Finally,since 2x =25,then Lisa earns $25per hour.2.(a)The perimeter of the region includes the diameter and the semi-circle.Since the radius of the region is 10,then the length of its diameter is 20.Since the radius of the region is 10,then the circumference of an entire circle with this radius is 2π(10)=20π,so the arc length of the semi-circle is one-half of 20π,or 10π.Therefore,the perimeter of the region is 10π+20.(b)The x -intercepts of the parabola with equation y =10(x +2)(x −5)are −2and 5.Since the line segment,P Q ,joining these points is horizontal,then its length is the diﬀerence in the intercepts,or 5−(−2)=7.(c)The slope of the line joining the points C (0,60)and D (30,0)is60−00−30=60−30=−2.Since this line passes through C (0,60),then the y -intercept of the line is 60,and so an equation of the line is y =−2x +60.We thus want to ﬁnd the point of intersection,E ,between the lines with equations y =−2x +60and y =2x .Equating y -coordinates,we obtain −2x +60=2x or 4x =60,and so x =15.Substituting x =15into the equation y =2x ,we obtain y =2(15)=30.Therefore,the coordinates of E are (15,30).3.(a)We note that BD =BC +CD and that BC =20cm,so we need to determine CD .We draw a line from C to P on F D so that CP is perpendicular to DF .Since AC and DF are parallel,then CP is also perpendicular toAC .The distance between AC and DF is 4cm,so CP =4cm.Since ABC is isosceles and right-angled,then ∠ACB =45◦.AD B CEF 204P Thus,∠P CD =180◦−∠ACB −∠P CA =180◦−45◦−90◦=45◦.Since CP D is right-angled at P and ∠P CD =45◦,then CP D is also an isosceles right-angled triangle.Therefore,CD =√2CP =4√2cm.Finally,BD =BC +CD =(20+4√2)cm.(b)Manipulating the given equation and noting that x =0and x =−12since neither denom-inator can equal 0,we obtainx 2+x +42x +1=4xx (x 2+x +4)=4(2x +1)x 3+x 2+4x =8x +4x 3+x 2−4x −4=0x 2(x +1)−4(x +1)=0(x +1)(x 2−4)=0(x +1)(x −2)(x +2)=Therefore,x =−1or x =2or x =−2.We can check by substitution that each satisﬁes the original equation.4.(a)Solution 1Since 900=302and 30=2×3×5,then 900=223252.The positive divisors of 900are those integers of the form d =2a 3b 5c ,where each of a,b,c is 0,1or 2.For d to be a perfect square,the exponent on each prime factor in the prime factorization of d must be even.Thus,for d to be a perfect square,each of a,b,c must be 0or 2.There are two possibilities for each of a,b,c so 2×2×2=8possibilities for d .These are 203050=1,223050=4,203250=9,203052=25,223250=36,223052=100,203252=225,and 223252=900.Thus,8of the positive divisors of 900are perfect squares.Solution 2The positive divisors of 900are1,2,3,4,5,6,9,10,12,15,18,20,25,30,36,45,50,60,75,90,100,150,180,225,300,450,900Of these,1,4,9,25,36,100,225,and 900are perfect squares (12,22,32,52,62,102,152,302,respectively).Thus,8of the positive divisors of 900are perfect squares.(b)In isosceles triangle ABC ,∠ABC =∠ACB ,so the sides opposite these angles (AC andAB ,respectively)are equal in length.Since the vertices of the triangle are A (k,3),B (3,1)and C (6,k ),then we obtainAC=AB(k −6)2+(3−k )2=(k −3)2+(3−1)2(k −6)2+(3−k )2=(k −3)2+(3−1)2(k −6)2+(k −3)2=(k −3)2+22(k −6)2=4Thus,k −6=2or k −6=−2,and so k =8or k =4.We can check by substitution that each satisﬁes the original equation.5.(a)Bottle A contains 40g of which 10%is acid.Thus,it contains 0.1×40=4g of acid and 40−4=36g of water.Bottle B contains 50g of which 20%is acid.Thus,it contains 0.2×50=10g of acid and 50−10=40g of water.Bottle C contains 50g of which 30%is acid.Thus,it contains 0.3×50=15g of acid and 50−15=35g of water.In total,the three bottles contain 40+50+50=140g,of which 4+10+15=29g is acid and 140−29=111g is water.The new mixture has mass 60g of which 25%is acid.Thus,it contains 0.25×60=15g of acid and 60−15=45g of water.Since the total mass in the three bottles is initially 140g and the new mixture has mass 60g,then the remaining contents have mass 140−60=80g.Since the total mass of acid in the three bottles is initially 29g and the acid in the new mixture has mass 15g,then the acid in the remaining contents has mass 29−15=14g.This remaining mixture is thus14g80g×100%=17.5%acid.(b)Since 3x +4y =10,then 4y =10−3x .Therefore,when 3x +4y =10,x 2+16y 2=x 2+(4y )2=x 2+(10−3x )2=x 2+(9x 2−60x +100)=10x 2−60x +100=10(x 2−6x +10)=10(x 2−6x +9+1)=10((x −3)2+1)=10(x −3)2+10Since (x −3)2≥0,then the minimum possible value of 10(x −3)2+10is 10(0)+10=10.This occurs when (x −3)2=0or x =3.Therefore,the minimum possible value of x 2+16y 2when 3x +4y =10is 10.6.(a)Solution 1Suppose that the bag contains g gold balls.We assume that Feridun reaches into the bag and removes the two balls one after the other.There are 40possible balls that he could remove ﬁrst and then 39balls that he could remove second.In total,there are 40(39)pairs of balls that he could choose in this way.If he removes 2gold balls,then there are g possible balls that he could remove ﬁrst and then g −1balls that he could remove second.In total,there are g (g −1)pairs of gold balls that he could remove.We are told that the probability of removing 2gold balls is 512.Since there are 40(39)total pairs of balls that can be chosen and g (g −1)pairs ofgold balls that can be chosen in this way,then g (g −1)40(39)=512which is equivalent tog (g −1)=512(40)(39)=650.Therefore,g 2−g −650=0or (g −26)(g +25)=0,and so g =26or g =−25.Since g >0,then g =26,so there are 26gold balls in the bag.Solution 2Suppose that the bag contains g gold balls.We assume that Feridun reaches into the bag and removes the two balls together.Since there are 40balls in the bag,there are 402pairs of balls that he could choose inthis way.Since there are g gold balls in the bag,then there are g2pairs of gold balls that he couldchoose in this way.We are told that the probability of removing 2gold balls is 512.Since there are 402 pairs in total that can be chosen and g2pairs of gold balls thatcan be chosen in this way,then g22 =512which is equivalent to g 2 =512 402.Since n 2=n (n −1)2,then this equation is equivalent to g (g −1)2=51240(39)2=325.Therefore,g (g −1)=650or g 2−g −650=0or (g −26)(g +25)=0,and so g =26or g =−25.Since g >0,then g =26,so there are 26gold balls in the bag.(b)Suppose that the ﬁrst term in the geometric sequence is t 1=a and the common ratio inthe sequence is r .Then the sequence,which has n terms,is a,ar,ar 2,ar 3,...,ar n −1.In general,the k th term is t k =ar k −1;in particular,the n th term is t n =ar n −1.Since t 1t n =3,then a ·ar n −1=3or a 2r n −1=3.Since t 1t 2···t n −1t n =59049,then(a )(ar )···(ar n −2)(ar n −1)=59049a n rr 2···r n −2r n −1=59049(since there are n factors of a on the left side)a n r 1+2+···+(n −2)+(n −1)=59049a n r 12(n −1)(n )=59049since 1+2+···+(n −2)+(n −1)=12(n −1)(n ).Since a 2r n −1=3,then (a 2r n −1)n =3n or a 2n r (n −1)(n )=3n .Since a n r 12(n −1)(n )=59049,then a n r 12(n −1)(n ) 2=590492or a 2n r (n −1)(n )=590492.Since the left sides of these equations are the same,then 3n =590492.Now59049=3(19683)=32(6561)=33(2187)=34(729)=35(243)=36(81)=3634=310Since 59049=310,then 590492=320and so 3n =320,which gives n =20.7.(a)Let a =x −2013and let b =y −2014.The given equation becomes ab a 2+b 2=−12,which is equivalent to 2ab =−a 2−b 2anda 2+2ab +b 2=0.This is equivalent to (a +b )2=0which is equivalent to a +b =0.Since a =x −2013and b =y −2014,then x −2013+y −2014=0or x +y =4027.(b)Let a =log 10x .Then (log 10x )log 10(log 10x )=10000becomes a log 10a =104.Taking the base 10logarithm of both sides and using the fact that log 10(a b )=b log 10a ,we obtain (log 10a )(log 10a )=4or (log 10a )2=4.Therefore,log 10a =±2and so log 10(log 10x )=±2.If log 10(log 10x )=2,then log 10x =102=100and so x =10100.If log 10(log 10x )=−2,then log 10x =10−2=1100and so x =101/100.Therefore,x =10100or x =101/100.We check these answers in the original equation.If x =10100,then log 10x =100.Thus,(log 10x )log 10(log 10x )=100log 10100=1002=10000.If x =101/100,then log 10x =1/100=10−2.Thus,(log 10x )log 10(log 10x )=(10−2)log 10(10−2)=(10−2)−2=104=10000.8.(a)We use the cosine law in ABD to determine the length of BD :BD 2=AB 2+AD 2−2(AB )(AD )cos(∠BAD )We are given that AB =75and AD =20,so we need to determine cos(∠BAD ).Nowcos(∠BAD )=cos(∠BAC +∠EAD )=cos(∠BAC )cos(∠EAD )−sin(∠BAC )sin(∠EAD )=AC AB AD AE −BC AB ED AEsince ABC and ADE are right-angled.Since AB =75and BC =21,then by the Pythagorean Theorem,AC =√AB 2−BC 2=√752−212=√5625−441=√5184=72since AC >0.Since AC =72and CE =47,then AE =AC −CE =25.Since AE =25and AD =20,then by the Pythagorean Theorem,ED =√AE 2−AD 2=√252−202=√625−400=√225=15since ED >0.Therefore,cos(∠BAD )=AC AB AD AE −BC AB ED AE =72752025−21751525=1440−31575(25)=112575(25)=4575=35BD 2=AB 2+AD 2−2(AB )(AD )cos(∠BAD )=752+202−2(75)(20)(35)=5625+400−1800=4225Since BD >0,then BD =√4225=65,as required.(b)Solution 1Consider BCE and ACD .ABCD EMNSince ABC is equilateral,then BC =AC .Since ECD is equilateral,then CE =CD .Since BCD is a straight line and ∠ECD =60◦,then ∠BCE =180◦−∠ECD =120◦.Since BCD is a straight line and ∠BCA =60◦,then ∠ACD =180◦−∠BCA =120◦.Therefore, BCE is congruent to ACD (“side-angle-side”).Since BCE and ACD are congruent and CM and CN are line segments drawn from the corresponding vertex (C in both triangles)to the midpoint of the opposite side,then CM =CN .Since ∠ECD =60◦,then ACD can be obtained by rotating BCE through an angle of 60◦clockwise about C .This means that after this 60◦rotation,CM coincides with CN .In other words,∠MCN =60◦.But since CM =CN and ∠MCN =60◦,then∠CMN =∠CNM =12(180◦−∠MCN )=60◦Therefore, MNC is equilateral,as required.We prove that MNC is equilateral by introducing a coordinate system.Suppose that C is at the origin (0,0)with BCD along the x -axis,with B having coordi-nates (−4b,0)and D having coordinates (4d,0)for some real numbers b,d >0.Drop a perpendicular from E to P on CD .Since ECD is equilateral,then P is the midpoint of CD .Since C has coordinates (0,0)and D has coordinates (4d,0),then the coordinates of P are (2d,0).Since ECD is equilateral,then ∠ECD =60◦and so EP C is a 30◦-60◦-90◦triangle and so EP =√3CP =2√3d .Therefore,the coordinates of E are (2d,2√3d ).In a similar way,we can show that the coordinates of A are (−2b,2√3b ).Now M is the midpoint of B (−4b,0)and E (2d,2√3d ),so the coordinates of M are 12(−4b +2d ),12(0+2√3d ) or (−2b +d,√3d ).Also,N is the midpoint of A (−2b,2√3b )and D (4d,0),so the coordinates of N are 12(−2b +4d ),12(2√3b +0) or (−b +2d,√3b ).To show that MNC is equilateral,we show that CM =CN =MN or equivalently that CM 2=CN 2=MN 2:CM 2=(−2b +d −0)2+(√3d −0)2=(−2b +d )2+(√d )2=4b 2−4bd +d 2+3d 2=4b 2−4bd +4d 2CN 2=(−b +2d −0)2+(√−0)2=(−b +2d )2+(√3b )2=b 2−4bd +4d 2+3b 2=4b 2−4bd +4d 2MN 2=((−2b +d )−(−b +2d ))2+(√3d −√3b )2=(−b −d )2+3(d −b )2=b 2+2bd +d 2+3d 2−6bd +3b 2=4b 2−4bd +4d 2Therefore,CM 2=CN 2=MN 2and so MNC is equilateral,as required.9.(a)Let S=sin61◦+sin62◦+sin63◦+···+sin687◦+sin688◦+sin689◦.Since sinθ=cos(90◦−θ),then sin6θ=cos6(90◦−θ),and soS=sin61◦+sin62◦+···+sin644◦+sin645◦+cos6(90◦−46◦)+cos6(90◦−47◦)+···+cos6(90◦−89◦)=sin61◦+sin62◦+···+sin644◦+sin645◦+cos644◦+cos643◦+···+cos61◦=(sin61◦+cos61◦)+(sin62◦+cos62◦)+···+(sin644◦+cos644◦)+sin645◦Since sin45◦=1√2,then sin645◦=123=18.Also,sincex3+y3=(x+y)(x2−xy+y2)=(x+y)((x+y)2−3xy)then substituting x=sin2θand y=cos2θ,we obtainx3+y3=(x+y)((x+y)2−3xy)sin6θ+cos6θ=(sin2θ+cos2θ)((sin2θ+cos2θ)2−3sin2θcos2θ)sin6θ+cos6θ=1(1−3sin2θcos2θ)since sin2θ+cos2θ=1.Therefore,S=(sin61◦+cos61◦)+(sin62◦+cos62◦)+···+(sin644◦+cos644◦)+sin645◦=(1−3sin21◦cos21◦)+(1−3sin22◦cos22◦)+···+(1−3sin244◦cos244◦)+18 =44−(3sin21◦cos21◦+3sin22◦cos22◦+···+3sin244◦cos244◦)+18=3538−34(4sin21◦cos21◦+4sin22◦cos22◦+···+4sin244◦cos244◦)Since sin2θ=2sinθcosθ,then4sin2θcos2θ=sin22θ,which gives S=353−3(4sin21◦cos21◦+4sin22◦cos22◦+···+4sin244◦cos244◦)=3538−34(sin22◦+sin24◦+···+sin288◦)=3538−34(sin22◦+sin24◦+···+sin244◦+sin246◦+···+sin286◦+sin288◦)=3538−34(sin22◦+sin24◦+···+sin244◦+cos2(90◦−46◦)+···+cos2(90◦−86◦)+cos2(90◦−88◦))=3538−34(sin22◦+sin24◦+···+sin244◦+cos244◦+···+cos24◦+cos22◦)=3538−34((sin22◦+cos22◦)+(sin24◦+cos24◦)+···+(sin244◦+cos244◦))=353−3(22)(since sin2θ+cos2θ=1)=3538−1328=2218Therefore,since S=mn,then m=221and n=8satisfy the required equation.(b)First,we prove that f(n)=n(n+1)(n+2)(n+3)24in two diﬀerent ways.Method1If an n-digit integer has digits with a sum of5,then there are several possibilities for the combination of non-zero digits used:54,13,23,1,12,2,12,1,1,11,1,1,1,1We count the number of possible integers in each case by determining the number of arrangements of the non-zero digits;we call the number of ways of doing this a.(For example,the digits4and1can be arranged as41or14.)We then place the leftmost digit in such an arrangement as the leftmost digit of the n-digit integer(which must be non-zero)and choose the positions for the remaining non-zero digits among the remaining n−1 positions;we call the number of ways of doing this b.(For example,for the arrangement 14,the digit1is in the leftmost position and the digit4can be in any of the remaining n−1positions.)Weﬁll the rest of the positions with0s.The number of possible integers in each case will be ab,since this method will create all such integers and for each of the a arrangements of the non-zero digits,there will be b ways of arranging the digits after theﬁrst one.We make a chart to summarize the cases,expanding each total and writing it as a fraction with denominator24:Case a b ab(expanded)5111=24 244,12(n−1)2(n−1)=48n−48243,22(n−1)2(n−1)=48n−48243,1,13n−123n−12=36n2−108n+72242,2,13n−123n−12=36n2−108n+72242,1,1,14n−134n−13=16n3−96n2+176n−96241,1,1,1,11n−14n−14=n4−10n3+35n2−50n+2424(Note that in the second and third cases we need n≥2,in the fourth andﬁfth cases we need n≥3,in the sixth case we need n≥4,and the seventh case we need n≥5.In each case,though,the given formula works for smaller positive values of n since it is equal to 0in each case.Note also that we say b=1in theﬁrst case since there is exactly1way of placing0s in all of the remaining n−1positions.)f(n)is then the sum of the expressions in the last column of this table,and sof(n)=n4+6n3+11n2+6n24=n(n+1)(n+2)(n+3)24as required.Method2First,we create a correspondence between each integer with n digits and whose digits havea sum of 5and an arrangement of ﬁve 1s and (n −1)Xs that begins with a 1.We can then count these integers by counting the arrangements.Starting with such an integer,we write down an arrangement of the above type using the following rule:The number of 1s to the left of the ﬁrst X is the ﬁrst digit of the number,thenumber of 1s between the ﬁrst X and second X is the second digit of the number,and so on,with the number of 1s to the right of the (n −1)st X representing then th digit of the number.For example,the integer 1010020001would correspond to 1XX1XXX11XXXX1.In this way,each such integer gives an arrangement of the above type.Similarly,each arrangement of this type can be associated back to a unique integer with the required properties by counting the number of 1s before the ﬁrst X and writing this down as the leftmost digit,counting the number of 1s between the ﬁrst and second Xs and writing this down as the second digit,and so on.Since a total of ﬁve 1s are used,then each arrangement corresponds with an integer with n digits whose digits have a sum of 5.Therefore,there is a one-to-one correspondence between the integers and arrangements with the desired properties.Thus,f (n ),which equals the number of such integers,also equals the number of such arrangements.To count the number of such arrangements,we note that there are four 1s and n −1Xs to arrange in the ﬁnal 4+(n −1)=n +3positions,since the ﬁrst position is occupied by a 1.There are n +34 ways to choose the positions of the remaining four 1s,and so n +34 arrangements.Thus,f (n )= n +34=(n +3)!4!(n −1)!=(n +3)(n +2)(n +1)(n )4!=n (n +1)(n +2)(n +3)24.Next,we need to determine the positive integers n between 1and 2014,inclusive,for which the units digit of f (n )is 1.Now f (n )=n (n +1)(n +2)(n +3)24is an integer for all positive integers n ,since it is counting the number of things with a certain property.If the units digit of n is 0or 5,then n is a multiple of 5.If the units digit of n is 2or 7,then n +3is a multiple of 5.If the units digit of n is 3or 8,then n +2is a multiple of 5.If the units digit of n is 4or 9,then n +1is a multiple of 5.Thus,if the units digit of n is 0,2,3,4,5,7,8,or 9,then n (n +1)(n +2)(n +3)is a multiple of 5and so f (n )=n (n +1)(n +2)(n +3)24is a multiple of 5,since the denominator contains no factors of 5that can divide the factor from the numerator.Therefore,if the units digit of n is 0,2,3,4,5,7,8,or 9,then f (n )is divisible by 5,and so cannot have a units digit of 1.So we consider the cases where n has a units digit of 1or of 6;these are the only possible values of n for which f (n )can have a units digit of 1.We note that 3f (n )=n (n +1)(n +2)(n +3)8,which is a positive integer for all positive integers n .Also,we note that if f(n)has units digit1,then3f(n)has units digit3,and if3f(n)has units digit3,then f(n)must have units digit1.Therefore,determining the values of n for which f(n)has units digit1is equivalent todetermining the values of n for which n(n+1)(n+2)(n+3)8has units digit3.We consider the integers n in groups of40.(Intuitively,we do this because the problem seems to involve multiples of5and multiples of8,and5×8=40.)If n has units digit1,then n=40k+1or n=40k+11or n=40k+21or n=40k+31 for some integer k≥0.If n has units digit6,then n=40k+6or n=40k+16or n=40k+26or n=40k+36 for some integer k≥0.If n=40k+1,then3f(n)=n(n+1)(n+2)(n+3)8=(40k+1)(40k+2)(40k+3)(40k+4)8=(40k+1)(20k+1)(40k+3)(10k+1)The units digit of40k+1is1,the units digit of20k+1is1,the units digit of40k+3is 3,and the units digit of10k+1is1,so the units digit of the product is the units digit of (1)(1)(3)(1)or3.In a similar way,we treat the remaining seven cases and summarize all eight cases in a chart:n3f(n)simpliﬁed Units digit of3f(n) 40k+1(40k+1)(20k+1)(40k+3)(10k+1)340k+11(40k+11)(10k+3)(40k+13)(20k+7)340k+21(40k+21)(20k+11)(40k+23)(10k+6)840k+31(40k+31)(10k+8)(40k+33)(20k+17)840k+6(20k+3)(40k+7)(10k+2)(40k+9)840k+16(10k+4)(40k+17)(20k+9)(40k+19)840k+26(20k+13)(40k+27)(10k+7)(40k+29)340k+36(10k+9)(40k+37)(20k+19)(40k+39)3(Note that,for example,when n=40k+16,the simpliﬁed version of3f(n)is (10k+4)(40k+17)(20k+9)(40k+19),so the units digit of3f(n)is the units digit of(4)(7)(9)(9)which is the units digit of2268,or8.)Therefore,f(n)has units digit1whenever n=40k+1or n=40k+11or n=40k+26 or n=40k+36for some integer k≥0.There are4such integers n between each pair of consecutive multiples of40.Since2000=50×40,then2000is the50th multiple of40,so there are50×4=200 integers n less than2000for which the units digit of f(n)is1.Between2000and2014,inclusive,there are two additional integers:n=40(50)+1=2001 and n=40(50)+11=2011.In total,202of the integers f(1),f(2),...,f(2014)have a units digit of1.10.Throughout this solution,we use“JB”to represent“jelly bean”or“jelly beans”.We use“T1”to represent“Type1move”,“T2”to represent“Type2move”,and so on.We use“P0”to represent“position0”,“P1”to represent“position1”,and so on.We represent the positions of the JB initially or after a move using an ordered tuple of non-negative integers representing the number of JB at P0,P1,P2,etc.For example,the tuple (0,0,1,2,1)would represent0JB at P0,0JB at P1,1JB at P2,2JB at P3,and1JB at P4.(a)To begin,we work backwards from theﬁnal state(0,0,0,0,0,1).The only move that could have put1JB at P5is1T5.Undoing this move removes1JB from P5and adds1JB at P4and1JB at P3,giving(0,0,0,1,1,0).The only move that could have put1JB at P4is1T4.Undoing this move removes1JB from P4and adds1JB at P2and1JB at P3,giving(0,0,1,2,0,0).The only moves that could put2JB at P3are2T3s.Undoing these moves removes2JB from P3,adds2JB at P1and2JB at P2,giving(0,2,3,0,0,0).The only moves that could put3JB at P2are3T2s.Undoing these moves gives(3,5,0,0,0,0).The only moves that could put5JB at P1are5T1s.Undoing these moves removes5JB from P1and adds10JB at P0,giving(13,0,0,0,0,0).Therefore,starting with N=13JB at P0allows Fiona to win the game by making all ofthe moves as above in the reverse order.In particular,from(13,0,0,0,0,0),5T1s gives(3,5,0,0,0,0),then3T2s give(0,2,3,0,0,0), then2T3s give(0,0,1,2,0,0),then1T4gives(0,0,0,1,1,0),then1T5gives(0,0,0,0,0,1), as required.(b)Initial Set-upFirst,we note that when Fiona starts with N JB(for someﬁxed positive integer N),thenthe gameﬁnishes in at most N−1moves(since she eats exactly one JB on each move).Second,we note that the positions of the JB in theﬁnal state as well as at any intermedi-ate state(that is,after some number of moves)must be in the list P0,P1,...,P(N−1),since each JB can move at most1position to the right on any given move,so no JB canmove more than N−1positions to the right in at most N−1moves.This means that,starting with N JB,any state can be described using an N-tuple(a0,a1,...,a N−2,a N−1),where a i represents the number of JB at P i in that state.Introduction of Fibonacci Sequence and Important Fact#1(IF1)We deﬁne the Fibonacci sequence by F1=1,F2=1,and F n=F n−1+F n−2for n≥3.The initial number of JB(N)and the number of JB at various positions are connectedusing the Fibonacci sequence in the following way.At any state between the starting state(N JB at P0)and theﬁnal state,if there are a iJB at P i for each i from0to N−1,thenN=a0F2+a1F3+···+a N−2F N+a N−1F N+1(∗)This is true because:•It is true for the starting state,since here(a0,a1,...,a N−2,a N−1)=(N,0,...,0,0)and F2=1,so the right side of(∗)equals N(1)+0or N•A T1does not change the value of the right side of(∗):Since a T1changes the state(a0,a1,a2,...,a N−2,a N−1)to(a0−2,a1+1,a2,...,a N−2,a N−1),the right side of(∗)changes froma0F2+a1F3+a2F4+···+a N−2F N+a N−1F N+1to(a0−2)F2+(a1+1)F3+a2F4+···+a N−2F N+a N−1F N+1 which is a diﬀerence of−2F2+F3=−2(1)+2=0.•A T i for i≥2does not change the value of the right side of(∗):Since a T i changes the state(a0,a1,...,a i−2,a i−1,a i,...,a N−2,a N−1)to(a0,a1,...,a i−2−1,a i−1−1,a i+1,...,a N−2,a N−1) the right side of(∗)changes froma0F2+a1F3+···+a i−2F i+a i−1F i+1+a i F i+2+···+a N−2F N+a N−1F N+1 toa0F2+a1F3+···+(a i−2−1)F i+(a i−1−1)F i+1+(a i+1)F i+2+···+a N−2F N+a N−1F N+1which is a diﬀerence of−F i−F i+1+F i+2=0since F i+2=F i+1+F i.This tells us that the value of the right side of(∗)starts at N and does not change on any subsequent move.Therefore,at any state(a0,a1,...,a N−2,a N−1)after starting with N JB at P0,it is true thatN=a0F2+a1F3+···+a N−2F N+a N−1F N+1(∗)To show that there is only one possibleﬁnal state when Fiona wins the game,we assume that there are two possible winningﬁnal states starting from N JB and show that these in fact must be the same state.Important Fact#2(IF2)To do this,we prove a property of Fibonacci numbers that will allow us to show that two sums of three or fewer non-consecutive Fibonacci numbers cannot be equal if the Fibonacci numbers used in each sum are not the same:If x,y,z are positive integers with2≤x<y<z and no pair of x,y,z are consecutive integers,then F z<F y+F z<F x+F y+F z<F z+1.Since each Fibonacci number is a positive integer,then F z<F y+F z<F x+F y+F z,so we must prove that F x+F y+F z<F z+1:Since no two of x,y,z are consecutive and x<y<z,then y<z−1.Since y and z are positive integers,then y≤z−2.Also,x<y−1≤z−3.Since x and z are integers with x<z−3,then x≤z−4.Since the Fibonacci sequence is increasing from F2onwards,thenF x+F y+F z≤F z−4+F z−2+F z<F z−3+F z−2+F z=F z−1+F z=F z+1Since there is a“<”in this chain of inequalities and equalities,then we obtain that F x+F y+F z<F z+1,as required.Completing the Proofof the problem that a winning state consists of three or fewer JB,each at a distinct position and no two at consecutive integer positions.Suppose that,starting from N JB at P0,in aﬁrst winningﬁnal state with a d=1,each of a b and a c equal to0or1and all other a i=0,and in a second winningﬁnal state with a D=1,each of a B and a C equal to0or1and all other a i=0.From IF1,this gives N=a b F b+2+a c F c+2+F d+2and N=a B F B+2+a C F C+2+F D+2,and so a b F b+2+a c F c+2+F d+2=a B F B+2+a C F C+2+F D+2.Starting from this last equation,we remove any common Fibonacci numbers from both sides.(Recall that each term on each side is either0or a Fibonacci number,and Fibonacci numbers on the same side are distinct.)If there are no Fibonacci numbers remaining on each side,then the winningﬁnal states are the same,as required.What happens if there are Fibonacci numbers remaining on either side?In this case,there must be Fibonacci numbers on each side,as otherwise we would have0equal to a non-zero number.Suppose that the largest Fibonacci number remaining on the LS is F k and the largest Fibonacci number remaining on the RS is F m.Since we have removed the common elements,then k=m,so we may assume that k<m; since k and m are integers,then k≤m−1.Note that the RS must be greater than or equal to F m,since it includes at least F m. Since the LS consists of at most three Fibonacci numbers,which are non-consecutive(since b,c,d are non-consecutive)and the largest of which is F k,then IF2tells us that the LS is less than F k+1.Since k+1≤m,then the LS is less than F m.Since the LS is less than F m and the RS is greater than or equal to F m,we have a contradiction,since they are supposed to be equal.Therefore,our assumption that Fibonacci numbers are left after removing the common numbers from each side is false.In other words,the positions of the JB in each of the winningﬁnal states are the same, so there is indeed only one possible winningﬁnal state.Therefore,if Fiona can win the game,then there is only one possibleﬁnal state.。

clmc加拿大数学竞赛例题

clmc加拿大数学竞赛例题题目：已知在三角形ABC中，AB = AC，且角BAC的度数为20°。

点D在边AC上，且AD = BC。

求角BDC的度数。

解题思路：1. 首先，由于AB = AC，我们知道三角形ABC是等腰三角形，因此角ABC = 角ACB。

2. 由于角BAC的度数为20°，我们可以计算出角ABC和角ACB的度数。

根据三角形内角和为180°，我们有：角ABC = 角ACB = (180°- 20°) / 2 = 80°3. 接下来，我们在三角形ABC外部构造一个等腰三角形ABE，使得AE = AB，且角BAE = 60°。

连接DE并延长交BC于点F。

4. 由于三角形ABE是等边三角形，我们有角ABE = 角AEB = 60°，且AB = AE = BE。

5. 由于AD = BC且AB = AC，我们可以得出角CAD = 角CBA。

由于角BAE = 60°，我们有角EAD = 角EAB -角BAC = 60°-20°= 40°。

6. 由于角EAD = 角CBA且AD = BC，我们可以得出三角形EAD 全等于三角形CBA（SAS）。

因此，ED = AB = AE。

7. 由于ED = AE，我们得出角AED = 角ADE = (180°- 40°) / 2 = 70°。

因此，角DEC = 180°- 70°= 110°。

8. 由于角DEC = 110°且角ACB = 80°，我们可以得出角BDC = 180°- 110°- 80°= 90°。

因此，角BDC的度数为90°。

请注意，上述题目及解题思路仅供参考，可能并不完全符合CLMC的题目难度和出题风格。

1、下载文档前请自行甄别文档内容的完整性，平台不提供额外的编辑、内容补充、找答案等附加服务。
2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
3、如文档侵犯您的权益，请联系客服反馈,我们会尽快为您处理(人工客服工作时间：9:00-18:30)。

1.For real numbers a and b with a≥0and b≥0,the operation is deﬁned bya b=√a+4b.For example,5 1=5+4(1)=√9=3.(a)What is the value of8 7?(b)If16 n=10,what is the value of n?(c)Determine the value of(9 18) 10.(d)With justiﬁcation,determine all possible values of k such that k k=k.2.Each week,the MathTunes Music Store releases a list of the Top200songs.A newsong“Recursive Case”is released in time to make it onto the Week1list.The song’s position,P,on the list in a certain week,w,is given by the equation P=3w2−36w+110.The week number w is always a positive integer.(a)What position does the song have on week1?(b)Artists want their song to reach the best position possible.The closer that theposition of a song is to position#1,the better the position.(i)What is the best position that the song“Recursive Case”reaches?(ii)On what week does this song reach its best position?(c)What is the last week that“Recursive Case”appears on the Top200list?3.A pyramid ABCDE has a square base ABCD of side length 20.Vertex E lies on theline perpendicular to the base that passes through F ,the centre of the base ABCD .It is given that EA =EB =EC =ED =18.(a)Determine the surface area of the pyramidABCDEincluding its base.(b)Determine the height EF of the pyramid.A B C D EF 2018(c)G and H are the midpoints of ED and EA ,respectively.Determine the area of thequadrilateral BCGH .4.The triple ofpositive integers (x,y,z )is called an Almost Pythagorean Triple (or APT)if x >1and y >1and x 2+y 2=z 2+1.For example,(5,5,7)is an APT.(a)Determine the values of y and z so that (4,y,z )is an APT.(b)Prove that for any triangle whose side lengths form an APT,the area of thetriangle is not an integer.(c)Determine two 5-tuples (b,c,p,q,r )of positive integers with p ≥100for which(5t +p,bt +q,ct +r )is an APT for all positive integers t .1.At the JK Mall grand opening,some lucky shoppers are able to participate in a moneygiveaway.A large box has beenﬁlled with many$5,$10,$20,and$50bills.The lucky shopper reaches into the box and is allowed to pull out one handful of bills.(a)Rad pulls out at least two bills of each type and his total sum of money is$175.What is the total number of bills that Rad pulled out?(b)Sandy pulls out exactlyﬁve bills and notices that she has at least one bill of eachtype.What are the possible sums of money that Sandy could have?(c)Lino pulls out six or fewer bills and his total sum of money is$160.There areexactly four possibilities for the number of each type of bill that Lino could have.Determine these four possibilities.2.A parabola has equation y=(x−3)2+1.(a)What are the coordinates of the vertex of the parabola?(b)A new parabola is created by translating the original parabola3units to the leftand3units up.What is the equation of the translated parabola?(c)Determine the coordinates of the point of intersection of these two parabolas.(d)The parabola with equation y=ax2+4,a<0,touches the parabola withequation y=(x−3)2+1at exactly one point.Determine the value of a.3.A sequence of m P’s and n Q’s with m>n is called non-predictive if there is some pointin the sequence where the number of Q’s counted from the left is greater than or equal to the number of P’s counted from the left.For example,if m=5and n=2the sequence PPQQPPP is non-predictive because in counting theﬁrst four letters from the left,the number of Q’s is equal to the number of P’s.Also,the sequence QPPPQPP is non-predictive because in counting theﬁrst letterfrom the left,the number of Q’s is greater than the number ofP’s.(a)If m=7and n=2,determine the number of non-predictive sequences that beginwith P.(b)Suppose that n=2.Show that for every m>2,the number of non-predictivesequences that begin with P is equal to the number of non-predictive sequences that begin with Q.(c)Determine the number of non-predictive sequences with m=10and n=3.4.(a)Twenty cubes,each with edge length1cm,are placed together in4rows of5.What isthe surface area of this rectangularprism?(b)A number of cubes,each with edge length1cm,are arranged to form a rectangularprism having height1cm and a surface area of180cm2.Determine the number of cubes in the rectangular prism.(c)A number of cubes,each with edge length1cm,are arranged to form a rectangularprism having length l cm,width w cm,and thickness1cm.A frame is formed byremoving a rectangular prism with thickness 1cm located k cm from each of the sides of the original rectangular prism,as shown. Each of l,w and k is a positive integer.If the frame has surface area532cm2,determine all possible values for l and w such that l≥w.l cmw cmk cmk cmk cmk cm1 cm1.Quadrilateral P QRS is constructed with QR =51,as shown.The diagonals of P QRS intersect at 90◦at point T ,such that P T =32and QT =24.322451P QRST (a)Calculate the length of P Q.(b)Calculate the area of P QR .(c)If QS :P R =12:11,determine the perimeter of quadrilateral P QRS .2.(a)Determine the value of (a +b )2,given that a 2+b 2=24and ab =6.(b)If (x +y )2=13and x 2+y 2=7,determine the value of xy .(c)If j +k =6and j 2+k 2=52,determine the value of jk .(d)If m 2+n 2=12and m 4+n 4=136,determine all possible values of mn .3.(a)Points M (12,14)and N (n,n 2)lie on theparabola with equation y =x 2,as shown.Determine the value of n such that∠MON =90◦.yx(b)Points A (2,4)and B (b,b 2)are the endpointsofa chord of the parabola with equationy =x 2,as shown.Determine the value of bso that ∠ABO =90◦.y x(c)Right-angled triangle P QR is inscribed inthe parabola with equation y =x 2,asshown.Points P,Q and R have coordinates(p,p 2),(q,q 2)and (r,r 2),respectively.If p ,qand r are integers,show that 2q +p +r =0.y x4.The positive divisors of 21are 1,3,7and 21.Let S (n )be the sum of the positive divisors of the positive integer n .For example,S (21)=1+3+7+21=32.(a)If p is an odd prime integer,ﬁnd the value of p such that S (2p 2)=2613.(b)The consecutive integers 14and 15have the property that S (14)=S (15).Determine all pairs of consecutive integers m and n such that m =2p and n =9q for prime integers p,q >3,and S (m )=S (n ).(c)Determine the number of pairs of distinct prime integers p and q ,each less than 30,with the property that S (p 3q )is not divisible by 24.。