数字集成电路:电路系统与设计(第二版) (1)
(整理)集成电路原理学习指南-第二版

沟道等效电阻
(1)与W/L反比,
(2)与电压有关,
(3)VDD大的时候较小(饱和工作区)
(4)VDD接近Vt的时候急剧增大
(5)一般使用工作区平均电阻
掌握
3.18
电阻的近似
平均电阻,并估算其误差(保守估计还是过估计)
掌握
3.19
结构电容
栅电容,覆盖电容
掌握
3.20
沟道电容
在不同工作区域的变化和原因,在阈值附近最小
f=Cext/Cint=Cext/γCg,尺寸决定电容,所以也是扇出尺寸,为工艺决定的系数,代表自电容与栅电容的关系
掌握
5.13
反相器链的最优尺寸设计
每一级为前后级的几何平均
扇出系数公式(5.35),公式(5.36)
掌握
5.14
最佳等效扇出
图5.21(pp 152),一般取4
掌握
5.15
上升下降时间对延时的影响
了解
3.26
电容估算
(1)栅电容,扩散电容大致相当(定义单位NMOS和PMOS的栅电容为C)
(2)它们随沟道宽度等比增加(kC)
(3)最小晶体管C值可初略估计为1fF/um宽度(65nm工艺,宽0.1um晶体管的C值约为0.1fF)
[Weste,4.3.2]
掌握
第四章导线
序号
概念
知识点和关键词
掌握程度
掌握
3.13
MOS IV特性
画出IV图,标出工作区,图3.24(pp 74)
掌握并会定性画图
3.14
手工分析的局限
在电阻区和过度区之间的区域偏差较大
了解
3.15
设计测试点验证IV
知道晶体管几个端口的电压,固定哪个,量哪个电流,可以提取以上列出的某个参数。
数字集成电路--电路、系统与设计(第二版)课后练习题 第六章 CMOS组合逻辑门的设计

Chapter 6 Problem Set
Chapter 6 PROBLEMS
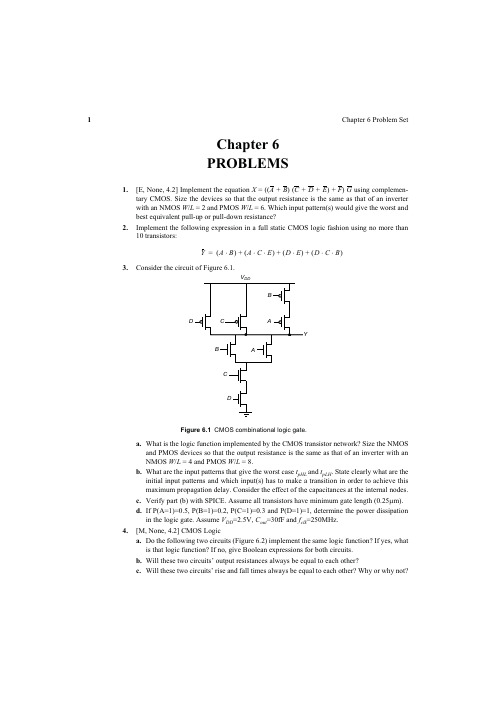
1. [E, None, 4.2] Implement the equation X = ((A + B) (C + D + E) + F) G using complementary CMOS. Size the devices so that the output resistance is the same as that of an inverter with an NMOS W/L = 2 and PMOS W/L = 6. Which input pattern(s) would give the worst and best equivalent pull-up or pull-down resistance? Implement the following expression in a full static CMOS logic fashion using no more than 10 transistors: Y = (A ⋅ B) + (A ⋅ C ⋅ E) + (D ⋅ E) + (D ⋅ C ⋅ B) 3. Consider the circuit of Figure 6.1.
2
VDD E 6 A A 6 B 6 C 6 D 6 E F A B C D 4 4 4 4 E 1 A B C D 4 4 4 4 E 1 6 F 6 B 6 C 6 D
Chapter 6 Problem Set
VDD 6
Circuit A
Circuit B
Figure 6.2 Two static CMOS gates.
数字集成电路-电路系统与设计第二版课程设计

数字集成电路-电路系统与设计第二版课程设计
一、课程设计介绍
数字集成电路是现代电路设计中的重要组成部分,也是计算机科学与工程的重要分支。
本课程设计旨在通过对数字集成电路的系统与设计进行探究,并结合具体的案例来设计和实现数字集成电路,使学生能够熟悉数字集成电路的基本原理、设计方法和实现技术。
本课程设计主要包含以下内容:
1.数值系统和编码
2.逻辑功能设计:组合逻辑电路和时序逻辑电路
3.集成电路设计方法和流程
4.VHDL和FPGA实现数字逻辑电路
5.数字信号处理器
通过本次课程设计,学生将掌握数字集成电路的系统性设计思路和实现方法,具备数字电路设计的基本能力和实际操作技术,能够针对具体应用场景提出解决方案,实现数字电路的设计、验证和调试。
二、课程设计要求
1. 课程设计题目
本次课程设计的题目为“4位计数器设计”。
2. 软件工具
VHDL编程软件和EDA工具
1。
【精品】数字集成电路电路、系统与设计第二版课后练习题第六章CMOS组合逻辑门的设计

【精品】数字集成电路--电路、系统与设计(第二版)课后练习题第六章CMOS组合逻辑门的设计第六章 CMOS组合逻辑门的设计1.为什么CMOS电路逻辑门的输入端和输出端都要连接到电源电压?CMOS电路采用了MOSFET(金属氧化物半导体场效应管)作为开关元件,其中N沟道MOSFET(NMOS)和P沟道MOSFET(PMOS)分别用于实现逻辑门的输入和输出。
NMOS和PMOS都需要连接到电源电压,以使其能够正常工作。
输入端连接到电源电压可以确保信号在逻辑门中正常传递,输出端连接到电源电压可以确保输出信号的正确性和稳定性。
2.为什么在CMOS逻辑门中要使用两个互补的MOSFET?CMOS逻辑门中使用两个互补的MOSFET是为了实现高度抗干扰的逻辑功能。
其中,NMOS和PMOS分别用于实现逻辑门的输入和输出。
NMOS和PMOS的工作原理互补,即当NMOS导通时,PMOS截止,当PMOS导通时,NMOS截止。
这样的设计可以在逻辑门的输出上提供高电平和低电平的稳定性,从而提高逻辑门的抗干扰能力。
3.CMOS逻辑门的输入电压范围是多少?CMOS逻辑门的输入电压范围通常是在0V至电源电压之间,即在低电平和高电平之间。
在CMOS逻辑门中,低电平通常定义为输入电压小于0.3Vdd(电源电压的30%),而高电平通常定义为输入电压大于0.7Vdd(电源电压的70%)。
4.如何设计一个基本的CMOS逻辑门?一个基本的CMOS逻辑门可以由一个NMOS和一个PMOS组成。
其中,NMOS的源极连接到地,栅极连接到逻辑门的输入,漏极连接到PMOS的漏极;PMOS的源极连接到电源电压,栅极连接到逻辑门的输入,漏极连接到输出。
这样的设计可以实现逻辑门的基本功能。
5.如何提高CMOS逻辑门的速度?可以采取以下方法来提高CMOS逻辑门的速度:•减小晶体管的尺寸:缩小晶体管的尺寸可以减小晶体管的电容和电阻,从而提高逻辑门的响应速度。
•优化电源电压:增加电源电压可以提高晶体管的驱动能力,从而加快逻辑门的开关速度。
数字集成电路--电路、系统与设计(第二版)课后练习题第六.
数字集成电路--电路、系统与设计(第⼆版)课后练习题第六.Digital Integrated Circuits - 2nd Ed 11 DESIGN PROJECT Design, lay out, and simulate a CMOS four-input XOR gate in the standard 0.25 micron CMOS process. You can choose any logic circuit style, and you are free to choose how many stages of logic to use: you could use one large logic gate or a combination of smaller logic gates. The supply voltage is set at 2.5 V! Your circuit must drive an external 20 fF load in addition to whatever internal parasitics are present in your circuit. The primary design objective is to minimize the propagation delay of the worst-case transition for your circuit. The secondary objective is to minimize the area of the layout. At the very worst, your design must have a propagation delay of no more than 0.5 ns and occupy an area of no more than 500 square microns, but the faster and smaller your circuit, the better. Be aware that, when using dynamic logic, the precharge time should be made part of the delay. The design will be graded on themagnitude of A × tp2, the product of the area of your design and the square of the delay for the worst-case transition.。
数字集成电路--电路、系统与设计

数字集成电路是现代电子产品中不可或缺的一部分,它们广泛应用于计算机、手机、汽车、医疗设备等领域。
数字集成电路通过在芯片上集成大量的数字电子元件,实现了电子系统的高度集成和高速运算。
本文将从电路、系统与设计三个方面探讨数字集成电路的相关内容。
一、数字集成电路的电路结构数字集成电路的电路结构主要包括逻辑门、寄存器、计数器等基本元件。
其中,逻辑门是数字集成电路中最基本的构建元件,包括与门、或门、非门等,通过逻辑门的组合可以实现各种复杂的逻辑功能。
寄存器是用于存储数据的元件,通常由触发器构成;而计数器则可以实现计数和计时功能。
这些基本的电路结构构成了数字集成电路的基础,为实现各种数字系统提供了必要的支持。
二、数字集成电路与数字系统数字集成电路是数字系统的核心组成部分,数字系统是以数字信号为处理对象的系统。
数字系统通常包括输入输出接口、控制单元、运算器、存储器等部分,数字集成电路在其中充当着处理和控制信号的角色。
数字系统的设计需要充分考虑数字集成电路的特性,包括时序和逻辑的正确性、面积和功耗的优化等方面。
数字集成电路的发展也推动了数字系统的不断完善和创新,使得数字系统在各个领域得到了广泛的应用。
三、数字集成电路的设计方法数字集成电路的设计过程通常包括需求分析、总体设计、逻辑设计、电路设计、物理设计等阶段。
需求分析阶段需要充分了解数字系统的功能需求,并将其转化为具体的电路规格。
总体设计阶段需要根据需求分析的结果确定电路的整体结构和功能分配。
逻辑设计阶段是将总体设计转化为逻辑电路图,其中需要考虑逻辑函数、时序关系、并行性等问题。
电路设计阶段是将逻辑电路图转化为电路级电路图,包括门电路的选择和优化等。
物理设计阶段则是将电路级电路图转化为实际的版图设计,考虑布线、功耗、散热等问题。
在每个设计阶段都需要充分考虑电路的性能、面积、功耗等指标,以实现设计的最优化。
结语数字集成电路作为现代电子系统的关键组成部分,对于数字系统的功能和性能起着至关重要的作用。
数字电子电路第二版电子课件第一章数字电路基础

§1—1 数字信号与数字电路
4
第一章 数字电路基础
当人们在超市购物结账付款时,收银员只要把条形码扫描器对准货物上 的条形码一扫,计算机屏幕上立刻就会显示该物品的价格。这是因为条形 码经扫描器扫描后,会产生相应的“数字信号”,经计算机处理后就可以 显示为货物的名称及价格等信息,进而可刷卡付款,打印付款收据。超市 自动收款设备如图所示。
非逻辑开关电路
44
第一章 数字电路基础
图所示为非门逻辑符号。非门真值表见表。 非门的逻辑功能可概括为“有0出1,有1出0”。非门的逻辑表达式为:
该表达式读作Y等于A非。
非门真值表
非门逻辑符号
45
28
第一章 数字电路基础
几种常见的BCD码
29
第一章 数字电路基础
(1)8421BCD码 最常用的BCD码是8421BCD码。 (2)5421BCD码 5421BCD码也是一种有权码,从高位到低位分别是5、4、2、1。 (3)2421BCD码 2421BCD码也是一种有权码,从高位到低位的权分别是2、4、2、1。 (4)余3码 这是一种无权码,它是在相应的8421BCD码上加0011(3)得到的。
15
第一章 数字电路基础
用数字电路测量电动机转速的原理框图
16
第一章 数字电路基础
2. 四人抢答器 四人抢答器原理框图如图所示。
四人抢答器原理框图
17
第一章 数字电路基础
从以上两个电路的工作过程可以看出,数字电路大致包含数字信号的产 生与整形、编码、寄存、译码、显示等典型单元数字电路。
此外,为了将传感器转换而来的模拟信号转换成控制系统所需要的数字 信号,必须采用模数转换器(A/D Converter)。数字信号被处理后,通常 还要经过数模转换器(D/A Converter)恢复成模拟信号,去驱动执行元件, 如图所示。
数字集成电路--电路、系统与设计(第二版)课后练习题-第四章 导线-Chapter 4 The Wire

1Chapter 4 Problem SetChapter 4Problems1.[M, None, 4.x] Figure 0.1 shows a clock-distribution network. Each segment of the clock net-work (between the nodes) is 5 mm long, 3 μm wide, and is implemented in polysilicon. Ateach of the terminal nodes (such as R ) resides a load capacitance of 100 fF.a.Determine the average current of the clock driver, given a voltage swing on the clock linesof 5 V and a maximum delay of 5 nsec between clock source and destination node R . Forthis part, you may ignore the resistance and inductance of the networkb.Unfortunately the resistance of the polysilicon cannot be ignored. Assume that eachstraight segment of the network can be modeled as a Π-network. Draw the equivalent cir-cuit and annotate the values of resistors and capacitors.c.Determine the dominant time-constant of the clock response at node R .2.[C, SPICE, 4.x] You are designing a clock distribution network in which it is critical to mini-mize skew between local clocks (CLK 1, CLK 2, and CLK 3). You have extracted the RC net-work of F igure 0.2, which models the routing parasitics of your clock line. Initially, you notice that the path to CLK 3 is shorter than to CLK 1 or CLK 2. In order to compensate for this imbalance, you insert a transmission gate in the path of CLK 3 to eliminate the skew.a.Write expressions for the time-constants associated with nodes CLK 1,CLK 2 and CLK 3.Assume the transmission gate can be modeled as a resistance R 3.b.If R 1 = R 2 = R 4 = R 5 = R and C 1 = C 2 = C 3 = C 4 = C 5 = C , what value of R 3 is required to balance the delays to CLK 1, CLK 2, and CLK 3?c.For R =750Ω and C =200fF, what (W /L )’s are required in the transmission gate to elimi-nate skew? Determine the value of the propagation delay.d.Simulate the network using SPICE, and compare the obtained results with the manually obtained numbers.3.[M, None, 4.x]Consider a CMOS inverter followed by a wire of length L . Assume that in thereference design, inverter and wire contribute equally to the total propagation delay t pref . Youmay assume that the transistors are velocity-saturated. The wire is scaled in line with the idealwire scaling model . Assume initially that the wire is a local wire .a.Determine the new (total) propagation delay as a a function of t p ref , assuming that technol-ogy and supply voltage scale with a factor 2. Consider only first-order effects.b.Perform the same analysis, assuming now that the wire scales a global wire , and the wire length scales inversely proportional to the technology.Figure 0.1Clock-distribution network.SR2Chapter 4 Problem Setc.Repeat b, but assume now that the wire is scaled along the constant resistance model. You may ignore the effect of the fringing capacitance.d.Repeat b, but assume that the new technology uses a better wiring material that reduces the resistivity by half, and a dielectric with a 25% smaller permittivity.e.Discuss the energy dissipation of part a. as a function of the energy dissipation of the orig-inal design E ref .f.Determine for each of the statements below if it is true, false, or undefined, and explain in one line your answer. - When driving a small fan-out, increasing the driver transistor sizes raises the short-circuit power dissipation. - Reducing the supply voltage, while keeping the threshold voltage constant decreases the short-circuit power dissipation.- Moving to Copper wires on a chip will enable us to build faster adders.- Making a wire wider helps to reduce its RC delay.- Going to dielectrics with a lower permittivity will make RC wire delay more impor-tant.4.[M, None, 4.x] A two-stage buffer is used to drive a metal wire of 1 cm. The first inverter is of minimum size with an input capacitance Ci=10 fF and an internal propagation delay t p0=50 ps and load dependent delay of 5ps/fF. The width of the metal wire is 3.6 μm. The sheet resis-tance of the metal is 0.08 Ω/, the capacitance value is 0.03 fF/μm 2and the fringing field capacitance is 0.04fF/μm.a.What is the propagation delay of the metal wire?pute the optimal size of the second inverter. What is the minimum delay through the buffer?c.If the input to the first inverter has 25% chance of making a 0-to-1 transition, and the whole chip is running at 20MHz with a 2.5 supply voltage, then what’s the power con-sumed by the metal wire?5.[M, None, 4.x]To connect a processor to an external memory an off -chip connection is neces-sary. The copper wire on the board is 15 cm long and acts as a transmission line with a charac-teristic impedance of 100Ω.(See F igure 0.3). The memory input pins present a very highimpedance which can be considered infinite. The bus driver is a CMOS inverter consisting ofvery large devices: (50/0.25) for the NMOS and (150/0.25) for the PMOS, where all sizes areClock CLK 1CLK 2CLK 3R 1R 2R 5R 4R 3Model as:Figure 0.2RC clock-distribution network.driver C 1C 3C 4C 5C 2Digital Integrated Circuits - 2nd Ed3 in μm. The minimum size device, (0.25/0.25) for NMOS and (0.75/0.25) for PMOS, has theon resistance 35 kΩ.a.Determine the time it takes for a change in the signal to propagate from source to destina-tion (time of flight). The wire inductance per unit length equals 75*10-8 H/m.b.Determine how long it will take the output signal to stay within 10% of its final value. Youcan model the driver as a voltage source with the driving device acting as a series resis-tance. Assume a supply and step voltage of 2.5V. Hint: draw the lattice diagram for thetransmission line.c.Resize the dimensions of the driver to minimize the total delay.L=15cmMemoryZ=100ΩFigure 0.3The driver, the connecting copper wire and thememory block being accessed.6.[M, None, 4.x] A two stage buffer is used to drive a metal wire of 1 cm. The first inverter is aminimum size with an input capacitance C i=10 fF and a propagation delay t p0=175 ps whenloaded with an identical gate. The width of the metal wire is 3.6 μm. The sheet resistance ofthe metal is 0.08 Ω/, the capacitance value is 0.03 fF/μm2 and the fringing field capacitanceis 0.04 fF/μm.a.What is the propagation delay of the metal wire?pute the optimal size of the second inverter. What is the minimum delay through thebuffer?7.[M, None, 4.x] For the RC tree given in Figure 0.4 calculate the Elmore delay from node A tonode B using the values for the resistors and capacitors given in the below in Table 0.1.Figure 0.4RC tree for calculating the delay4Chapter 4 Problem SetTable 0.1Values of the components in the RC tree of Figure 0.4Resistor Value(Ω)Capacitor Value(fF)R10.25C1250R20.25C2750R30.50C3250R4100C4250R50.25C51000R6 1.00C6250R70.75C7500R81000C82508.[M, SPICE, 4.x] In this problem the various wire models and their respective accuracies willbe studied.pute the 0%-50% delay of a 500um x 0.5um wire with resistance of 0.08 Ω/,witharea capacitance of 30aF/um2, and fringing capacitance of 40aF/um. Assume the driverhas a 100Ω resistance and negligible output capacitance.•Using a lumped model for the wire.•Using a PI model for the wire, and the Elmore equations to find tau. (see Chapter 4, figure4.26).•Using the distributed RC line equations from Chapter 4, section 4.4.4.pare your results in part a. using spice (be sure to include the source resistance). Foreach simulation, measure the 0%-50% time for the output•First, simulate a step input to a lumped R-C circuit.•Next, simulate a step input to your wire as a PI model.•Unfortunately, our version of SPICE does not support the distributed RC model as described in your book (Chapter 4, section 4.5.1). Instead, simulate a step input to yourwire using a PI3 distributed RC model.9.[M, None, 4.x] A standard CMOS inverter drives an aluminum wire on the first metal layer.Assume Rn=4kΩ, Rp=6kΩ. Also, assume that the output capacitance of the inverter is negli-gible in comparison with the wire capacitance. The wire is .5um wide, and the resistivity is0.08 Ω/..a.What is the "critical length" of the wire?b.What is the equivalent capacitance of a wire of this length? (For your capacitance calcula-tions, use Table 4.2 of your book , assume there’s field oxide underneath and nothingabove the aluminum wire)Digital Integrated Circuits - 2nd Ed510.[M, None, 4.x] A 10cm long lossless transmission line on a PC board (relative dielectric con-stant = 9, relative permeability = 1) with characteristic impedance of 50Ω is driven by a 2.5Vpulse coming from a source with 150Ω resistance.a.If the load resistance is infinite, determine the time it takes for a change at the source toreach the load (time of flight).Now a 200Ω load is attached at the end of the transmission line.b.What is the voltage at the load at t = 3ns?c.Draw lattice diagram and sketch the voltage at the load as a function of time. Determinehow long does it take for the output to be within 1 percent of its final value.11.[C, SPICE, 4.x] Assume V DD =1.5V . Also, use short-channel transistor models forhand analy-sis.a.The Figure 0.5 shows an output driver feeding a 0.2 pF effective fan-out of CMOS gates through a transmission line. Size the two transistors of the driver to optimize the delay.Sketch waveforms of V S and V L , assuming a square wave input. Label critical voltages and times.b.Size down the transistors by m times (m is to be treated as a parameter). Derive a first order expression for the time it takes for V L to settle down within 10% of its final voltage pare the obtained result with the case where no inductance is associated with the wire.Please draw the waveforms of V L for both cases, and comment.e the transistors as in part a). Suppose C L is changed to 20pF. Sketch waveforms of V S and V L , assuming a square wave input. Label critical voltages and instants.d.Assume now that the transmission line is lossy. Perform Hspice simulation for three cases:R=100 Ω/cm; R=2.5 Ω/cm; R=0.5 Ω/cm. Get the waveforms of V S , V L and the middle point of the line. Discuss the results.12.[M, None, 4.x] Consider an isolated 2mm long and 1μm wide M1(Metal1)wire over a silicon substrate driven by an inverter that has zero resistance and parasitic output capccitance. How will the wire delay change for the following cases? Explain your reasoning in each case.a.If the wire width is doubled.b.If the wire length is halved.c.If the wire thickness is doubled.d.If thickness of the oxide between the M1 and the substrate is doubled.13.[E, None, 4.x] In an ideal scaling model, where all dimensions and voltages scale with a fac-tor of S >1 :L=350nH/m 10cm C=150pF/m inV DDV DD V S V LC L =0.2pF Figure 0.5Transmission line between two inverters6Chapter 4 Problem Seta.How does the delay of an inverter scale?b.If a chip is scaled from one technology to another where all wire dimensions,including thevertical one and spacing, scale with a factor of S, how does the wire delayscale? How doesthe overall operating frequency of a chip scale?c.Repeat b) for the case where everything scales, except the vertical dimension of wires (itstays constant).。
数字集成电路--电路、系统与设计(第二版)复习资料

第一章 数字集成电路介绍第一个晶体管,Bell 实验室,1947第一个集成电路,Jack Kilby ,德州仪器,1958 摩尔定律:1965年,Gordon Moore 预言单个芯片上晶体管的数目每18到24个月翻一番。
(随时间呈指数增长)抽象层次:器件、电路、门、功能模块和系统 抽象即在每一个设计层次上,一个复杂模块的内部细节可以被抽象化并用一个黑匣子或模型来代替。
这一模型含有用来在下一层次上处理这一模块所需要的所有信息。
固定成本(非重复性费用)与销售量无关;设计所花费的时间和人工;受设计复杂性、设计技术难度以及设计人员产出率的影响;对于小批量产品,起主导作用。
可变成本 (重复性费用)与产品的产量成正比;直接用于制造产品的费用;包括产品所用部件的成本、组装费用以及测试费用。
每个集成电路的成本=每个集成电路的可变成本+固定成本/产量。
可变成本=(芯片成本+芯片测试成本+封装成本)/最终测试的成品率。
一个门对噪声的灵敏度是由噪声容限NM L (低电平噪声容限)和NM H (高电平噪声容限)来度量的。
为使一个数字电路能工作,噪声容限应当大于零,并且越大越好。
NM H = V OH - V IH NM L = V IL - V OL 再生性保证一个受干扰的信号在通过若干逻辑级后逐渐收敛回到额定电平中的一个。
一个门的VTC 应当具有一个增益绝对值大于1的过渡区(即不确定区),该过渡区以两个有效的区域为界,合法区域的增益应当小于1。
理想数字门 特性:在过渡区有无限大的增益;门的阈值位于逻辑摆幅的中点;高电平和低电平噪声容限均等于这一摆幅的一半;输入和输出阻抗分别为无穷大和零。
传播延时、上升和下降时间的定义传播延时tp 定义了它对输入端信号变化的响应有多快。
它表示一个信号通过一个门时所经历的延时,定义为输入和输出波形的50%翻转点之间的时间。
上升和下降时间定义为在波形的10%和90%之间。
对于给定的工艺和门的拓扑结构,功耗和延时的乘积一般为一常数。
数字集成电路:电路系统与设计(第二版)

数字集成电路:电路系统与设计(第二版)简介《数字集成电路:电路系统与设计(第二版)》是一本介绍数字集成电路的基本原理和设计方法的教材。
本书的内容覆盖了数字电路的基础知识、逻辑门电路、组合逻辑电路、时序逻辑电路、存储器和程序控制电路等方面。
通过学习本书,读者可以了解数字集成电路的概念、设计方法和实际应用。
目录1.数字电路基础知识 1.1 数字电路的基本概念 1.2 二进制系统与数制转换 1.3 逻辑运算与布尔代数2.逻辑门电路 2.1 与门、或门、非门 2.2 与非门、或非门、异或门 2.3 多输入门电路的设计方法3.组合逻辑电路 3.1 组合逻辑电路的基本原理 3.2 组合逻辑电路的设计方法 3.3 编码器和译码器4.时序逻辑电路 4.1 时序逻辑电路的基本原理 4.2 同步时序电路的设计方法 4.3 异步时序电路的设计方法5.存储器电路 5.1 存储器的基本概念 5.2 可读写存储器的设计方法 5.3 只读存储器的设计方法6.程序控制电路 6.1 程序控制电路的基本概念 6.2 程序控制电路的设计方法 6.3 微程序控制器的设计方法内容概述1. 数字电路基础知识本章主要介绍数字电路的基本概念,包括数字电路与模拟电路的区别、数字信号的表示方法以及数制转换等内容。
此外,还介绍了数字电路中常用的逻辑运算和布尔代数的基本原理。
2. 逻辑门电路逻辑门电路是数字电路中的基本组成单元,本章主要介绍了与门、或门、非门以及与非门、或非门、异或门等逻辑门的基本原理和组成。
此外,还介绍了多输入门电路的设计方法,以及逻辑门电路在数字电路设计中的应用。
3. 组合逻辑电路组合逻辑电路是由逻辑门电路组成的,本章主要介绍了组合逻辑电路的基本原理和设计方法。
此外,还介绍了编码器和译码器的原理和应用,以及在数字电路设计中的实际应用场景。
4. 时序逻辑电路时序逻辑电路是在组合逻辑电路的基础上引入了时序元件并进行时序控制的电路。
本章主要介绍了时序逻辑电路的基本原理和设计方法,包括同步时序电路和异步时序电路的设计。
数字集成电路—电路、系统与设计

数字集成电路(IC)在当今的电子装置和系统中发挥着至关重要的作用。
这些电路的设计将大量电子组件集成到一个单一芯片上,提供高性能和紧凑的尺寸。
在本篇文章中,我们将探索数字IC设计的关键方面,侧重于电路,系统和设计方面。
我们探索数字IC的电路方面。
数字 IC由晶体管,电阻器,电容器等基本电子元件构建而成,这些电子元件相互连接,可以实现逻辑功能。
现代数字IC集成水平惊人,数十亿晶体管被包装成一个芯片。
这种密集的集成使得在很小的物理空间内可以执行复杂的功能,如微处理器,内存单元,以及通信接口。
数字IC还设计为高速运行,消耗最小功率。
实现高速运行需要仔细考虑信号传播延迟,交叉对讲,以及动力消散。
为了应对这些挑战,IC设计师采用了先进的电路设计技术,如管道衬线,时钟标注,以及动力标注,以优化数字电路的性能和能效。
转到系统方面,数字IC常是更大的电子系统的一部分,它们与其他组件如传感器、起动器和通信接口相互作用。
数字IC的设计必须考虑到系统层面的要求,包括与外部组件的接口,处理输入、输出信号,以及支持各种通信协议。
数字IC在系统层面设计中的一个有趣例子是汽车电子领域。
现代车辆配备了广泛的数字IC,控制发动机,传输,安全系统,以及信息娱乐等功能。
这些IC必须满足可靠性、性能和安全性的严格要求,同时与各种传感器和起动器接口。
汽车数字IC的设计不仅涉及电路层面的考虑,还涉及系统层面的方面,如故障耐受性,通信协议,以及实时操作。
让我们谈谈数字IC的设计方面。
IC设计开始于具体说明电路的功能,之后是建筑和逻辑设计,电路执行,以及验证。
设计过程涉及各种工具和技术,包括逻辑综合、地点和路线、时间分析和功能核查。
设计可制造性和可检验性是关键考虑因素,可确保能够大规模生产高产量的IC并测试其可靠性。
IC设计中一个有趣的例子是开发适用于加密货币开采的集成电路。
为此目的设计的ASIC高度优化,用于履行采矿所需的密码散列功能,与一般用途处理器相比,往往能达到更高的性能和能源效率。
《集成电路设计(第2版)》习题答案1-5章

CH3
1. 写出晶体外延的意义,列出三种外延生长方法,并比较各自的优缺点。 意义:用同质材料形成具有不同掺杂种类及浓度而具有不同性能的晶体层。外延方法: 液态生长,气相外延生长,金属有机物气相外延生长 2.写出掩膜在 IC 制造过程中的作用,比较整版掩膜和单片掩膜的区别,列举三种掩膜的制 造方法。P28,29 3.写出光刻的作用,光刻有哪两种曝光方式? 作用: 把掩膜上的图形转换成晶圆上的器 件结构。曝光方式有接触与非接触两种。 4.X 射线制版和直接电子束直写技术替代光刻技术有什么优缺点? X 射线(X-ray)具有比可见光短得多的波长,可用来制作更高分辨率的掩膜版。电子
影响,界面势阱的影响 6. 什么是 MOS 器件的体效应? 由于衬底与源端未连接在一起,而引起的阈值电压的变化叫做体效应。 7. 说明 L、W 对 MOSFET 的速度、功耗、驱动能力的影响。 P70,71 8. MOSFET 按比例收缩后对器件特性有什么影响?
I DS
不变,器件占用面积减少,提高电路集成度,减少功耗
CH1
1. 按规模划分,集成电路的发展已经经历了哪几代?它的发展遵循了一条业界著名的定 律,请说出是什么定律? 晶体管-分立元件-SSI-MSI-LSI-VLSI-ULSI-GSI-SOC。MOORE 定律 2. 什么是无生产线集成电路设计?列出无生产线集成电路设计的特点和环境。 拥有设计人才和技术,但不拥有生产线。特点:电路设计,工艺制造,封装分立运行。 环境:IC 产业生产能力剩余,人们需要更多的功能芯片设计 3. 多项目晶圆(MPW)技术的特点是什么?对发展集成电路设计有什么意义? MPW:把几到几十种工艺上兼容的芯片拼装到一个宏芯片上,然后以步行的方式排列 到一到多个晶圆上。意义:降低成本。 4. 集成电路设计需要哪四个方面的知识? 系统,电路,工具,工艺方面的知识
数字集成电路--电路、系统与设计(第二版)复习资料

第一章 数字集成电路介绍第一个晶体管,Bell 实验室,1947第一个集成电路,Jack Kilby ,德州仪器,1958 摩尔定律:1965年,Gordon Moore 预言单个芯片上晶体管的数目每18到24个月翻一番。
(随时间呈指数增长)抽象层次:器件、电路、门、功能模块和系统 抽象即在每一个设计层次上,一个复杂模块的内部细节可以被抽象化并用一个黑匣子或模型来代替。
这一模型含有用来在下一层次上处理这一模块所需要的所有信息。
固定成本(非重复性费用)与销售量无关;设计所花费的时间和人工;受设计复杂性、设计技术难度以及设计人员产出率的影响;对于小批量产品,起主导作用。
可变成本 (重复性费用)与产品的产量成正比;直接用于制造产品的费用;包括产品所用部件的成本、组装费用以及测试费用。
每个集成电路的成本=每个集成电路的可变成本+固定成本/产量。
可变成本=(芯片成本+芯片测试成本+封装成本)/最终测试的成品率。
一个门对噪声的灵敏度是由噪声容限NM L (低电平噪声容限)和NM H (高电平噪声容限)来度量的。
为使一个数字电路能工作,噪声容限应当大于零,并且越大越好。
NM H = V OH - V IH NM L = V IL - V OL 再生性保证一个受干扰的信号在通过若干逻辑级后逐渐收敛回到额定电平中的一个。
一个门的VTC 应当具有一个增益绝对值大于1的过渡区(即不确定区),该过渡区以两个有效的区域为界,合法区域的增益应当小于1。
理想数字门 特性:在过渡区有无限大的增益;门的阈值位于逻辑摆幅的中点;高电平和低电平噪声容限均等于这一摆幅的一半;输入和输出阻抗分别为无穷大和零。
传播延时、上升和下降时间的定义传播延时tp 定义了它对输入端信号变化的响应有多快。
它表示一个信号通过一个门时所经历的延时,定义为输入和输出波形的50%翻转点之间的时间。
上升和下降时间定义为在波形的10%和90%之间。
对于给定的工艺和门的拓扑结构,功耗和延时的乘积一般为一常数。
数字集成电路--电路、系统与设计(第二版)课后练习题 第五章 CMOS反相器

C H A P T E R5T H E C M O S I N V E R T E R Quantification of integrity,performance,and energy metrics of an inverterOptimization of an inverter design5.1Exercises and Design Problems5.2The Static CMOS Inverter—An IntuitivePerspective5.3Evaluating the Robustness of the CMOSInverter:The Static Behavior5.3.1Switching Threshold5.3.2Noise Margins5.3.3Robustness Revisited5.4Performance of CMOS Inverter:The DynamicBehavior5.4.1Computing the Capacitances5.4.2Propagation Delay:First-OrderAnalysis5.4.3Propagation Delay from a DesignPerspective5.5Power,Energy,and Energy-Delay5.5.1Dynamic Power Consumption5.5.2Static Consumption5.5.3Putting It All Together5.5.4Analyzing Power Consumption UsingSPICE5.6Perspective:Technology Scaling and itsImpact on the Inverter Metrics180Section 5.1Exercises and Design Problems 1815.1Exercises and Design Problems1.[M,SPICE,3.3.2]The layout of a static CMOS inverter is given in Figure 5.1.(λ=0.125µm).a.Determine the sizes of the NMOS and PMOS transistors.b.Plot the VTC (using HSPICE)and derive its parameters (V OH ,V OL ,V M ,V IH ,and V IL ).c.Is the VTC affected when the output of the gates is connected to the inputs of 4similargates?.d.Resize the inverter to achieve a switching threshold of approximately 0.75V .Do not lay-out the new inverter,use HSPICE for your simulations.How are the noise margins affected by this modification?2.Figure 5.2shows a piecewise linear approximation for the VTC.The transition region isapproximated by a straight line with a slope equal to the inverter gain at V M .The intersectionof this line with the V OH and the V OL lines defines V IH and V IL .a.The noise margins of a CMOS inverter are highly dependent on the sizing ratio,r =k p /k n ,of the NMOS and PMOS e HSPICE with V Tn =|V Tp |to determine the valueof r that results in equal noise margins?Give a qualitative explanation.b.Section 5.3.2of the text uses this piecewise linear approximation to derive simplifiedexpressions for NM H and NM L in terms of the inverter gain.The derivation of the gain isbased on the assumption that both the NMOS and the PMOS devices are velocity saturatedat V M .For what range of r is this assumption valid?What is the resulting range of V M ?c.Derive expressions for the inverter gain at V M for the cases when the sizing ratio is justabove and just below the limits of the range where both devices are velocity saturated.What are the operating regions of the NMOS and the PMOS for each case?Consider theeffect of channel-length modulation by using the following expression for the small-signalresistance in the saturation region:r o,sat =1/(λI D ).Figure 5.1CMOS inverter layout.InOutGND V DD =2.5V.Poly Metal1NMOSPMOSPolyMetal12λ182THE CMOS INVERTER Chapter 53.[M,SPICE,3.3.2]Figure 5.3shows an NMOS inverter with resistive load.a.Qualitatively discuss why this circuit behaves as an inverter.b.Find V OH and V OL calculate V IH and V IL .c.Find NM L and NM H ,and plot the VTC using HSPICE.d.Compute the average power dissipation for:(i)V in =0V and (ii)V in =2.5Ve HSPICE to sketch the VTCs for R L =37k,75k,and 150k on a single graph.ment on the relationship between the critical VTC voltages (i.e.,V OL ,V OH ,V IL ,V IH )and the load resistance,R L .g.Do high or low impedance loads seem to produce more ideal inverter characteristics?4.[E,None,3.3.3]For the inverter of Figure 5.3and an output load of 3pF:a.Calculate t plh ,t phl ,and t p .b.Are the rising and falling delays equal?Why or why not?pute the static and dynamic power dissipation assuming the gate is clocked as fast as possible.5.The next figure shows two implementations of MOS inverters.The first inverter uses onlyNMOS transistors.V OH V OL inV outFigure 5.2A different approach to derive V IL and V IH .V outV in M 1W/L =1.5/0.5+2.5VFigure 5.3Resistive-load inverterR L =75k ΩSection 5.1Exercises and Design Problems183a.Calculate V OH ,V OL ,V M for each case.e HSPICE to obtain the two VTCs.You must assume certain values for the source/drain areas and perimeters since there is no layout.For our scalable CMOS process,λ =0.125μm,and the source/drain extensions are 5λfor the PMOS;for the NMOS the source/drain contact regions are 5λx5λ.c.Find V IH ,V IL ,NM L and NM H for each inverter and comment on the results.How can you increase the noise margins and reduce the undefined region?ment on the differences in the VTCs,robustness and regeneration of each inverter.6.Consider the following NMOS inverter.Assume that the bulk terminals of all NMOS deviceare connected to GND.Assume that the input IN has a 0V to 2.5V swing.a.Set up the equation(s)to compute the voltage on node x .Assume γ=0.5.b.What are the modes of operation of device M2?Assume γ=0.c.What is the value on the output node OUT for the case when IN =0V?Assume γ=0.d.Assuming γ=0,derive an expression for the switching threshold (V M )of the inverter.Recall that the switching threshold is the point where V IN =V OUT .Assume that the devicesizes for M1,M2and M3are (W/L)1,(W/L)2,and (W/L)3respectively.What are the limitson the switching threshold?For this,consider two cases:i)(W/L)1>>(W/L)2V DD =2.5V V IN V OUTV DD =2.5V V IN V OUT M 2M 1M 4M 3W/L=0.375/0.25W/L=0.75/0.25W/L=0.375/0.25W/L=0.75/0.25Figure 5.4Inverter ImplementationsV DD =2.5V OUTM1IN M2M3V DD =2.5Vx184THE CMOS INVERTER Chapter 5ii)(W/L)2>>(W/L)17.Consider the circuit in Figure 5.5.Device M1is a standard NMOS device.Device M2has allthe same properties as M1,except that its device threshold voltage is negative and has a valueof -0.4V.Assume that all the current equations and inequality equations (to determine themode of operation)for the depletion device M2are the same as a regular NMOS.Assume thatthe input IN has a 0V to 2.5V swing.a.Device M2has its gate terminal connected to its source terminal.If V IN =0V ,what is the output voltage?In steady state,what is the mode of operation of device M2for this input?pute the output voltage for V IN =2.5V .You may assume that V OUT is small to simplify your calculation.In steady state,what is the mode of operation of device M2for this input?c.Assuming Pr (IN =0)=0.3,what is the static power dissipation of this circuit?8.[M,None,3.3.3]An NMOS transistor is used to charge a large capacitor,as shown in Figure5.6.a.Determine the t pLH of this circuit,assuming an ideal step from 0to 2.5V at the input node.b.Assume that a resistor R S of 5k Ωis used to discharge the capacitance to ground.Deter-mine t pHL .c.Determine how much energy is taken from the supply during the charging of the capacitor.How much of this is dissipated in M1.How much is dissipated in the pull-down resistanceduring discharge?How does this change when R S is reduced to 1k Ω.d.The NMOS transistor is replaced by a PMOS device,sized so that k p is equal to the k n ofthe original NMOS.Will the resulting structure be faster?Explain why or why not.9.The circuit in Figure 5.7is known as the source follower configuration.It achieves a DC levelshift between the input and the output.The value of this shift is determined by the current I 0.Assume x d =0,γ=0.4,2|φf |=0.6V ,V T 0=0.43V ,k n ’=115μA/V 2and λ=0.V DD =2.5VOUTM1(4μm/1μm)IN M2(2μm/1μm),V Tn =-0.4VFigure 5.5A depletion load NMOSinverterV DD =2.5VOutFigure 5.6Circuit diagram with annotated W/L ratios=5pFSection 5.1Exercises and Design Problems 185a.Suppose we want the nominal level shift between V i and V o to be 0.6V in the circuit in Figure 5.7(a).Neglecting the backgate effect,calculate the width of M2to provide this level shift (Hint:first relate V i to V o in terms of I o ).b.Now assume that an ideal current source replaces M2(Figure 5.7(b)).The NMOS transis-tor M1experiences a shift in V T due to the backgate effect.Find V T as a function of V o for V o ranging from 0to 2.5V with 0.5V intervals.Plot V T vs.V oc.Plot V o vs.V i as V o varies from 0to 2.5V with 0.5V intervals.Plot two curves:one neglecting the body effect and one accounting for it.How does the body effect influence the operation of the level converter?d.At V o (with body effect)=2.5V,find V o (ideal)and thus determine the maximum error introduced by the body effect.10.For this problem assume:V DD =2.5V ,W P /L =1.25/0.25,W N /L =0.375/0.25,L =L eff =0.25μm (i.e.x d =0μm),C L =C inv-gate ,k n ’=115μA/V 2,k p ’=-30μA/V 2,V tn0=|V tp0|=0.4V,λ =0V -1, γ=0.4,2|φf |=0.6V ,and t ox =e the HSPICE model parameters for parasitic capacitance given below (i.e.C gd0,C j ,C jsw ),and assume that V SB =0V for all problems except part (e).Figure 5.7NMOS source follower configuration V DD =2.5V V iV oV DD =2.5VV i V oV bias =(a)(b)I o1um/0.25um M1186THE CMOS INVERTER Chapter 5##Parasitic Capacitance Parameters (F/m)##NMOS:CGDO=3.11x10-10,CGSO=3.11x10-10,CJ=2.02x10-3,CJSW=2.75x10-10PMOS:CGDO=2.68x10-10,CGSO=2.68x10-10,CJ=1.93x10-3,CJSW=2.23x10-10a.What is the V m for this inverter?b.What is the effective load capacitance C Leff of this inverter?(include parasitic capacitance,refer to the text for K eq and m .)Hint:You must assume certain values for the source/drain areas and perimeters since there is no layout.For our scalable CMOS process,λ =0.125μm,and the source/drain extensions are 5λfor the PMOS;for the NMOS the source/drain contact regions are 5λx5λ.c.Calculate t PHL ,t PLH assuming the result of (b)is ‘C Leff =6.5fF’.(Assume an ideal step input,i.e.t rise =t fall =0.Do this part by computing the average current used to charge/dis-charge C Leff .)d.Find (W p /W n )such that t PHL =t PLH .e.Suppose we increase the width of the transistors to reduce the t PHL ,t PLH .Do we get a pro-portional decrease in the delay times?Justify your answer.f.Suppose V SB =1V,what is the value of V tn ,V tp ,V m ?How does this qualitatively affect C Leff ?ing Hspice answer the following questions.a.Simulate the circuit in Problem 10and measure t P and the average power for input V in :pulse(0V DD 5n 0.1n 0.1n 9n 20n),as V DD varies from 1V -2.5V with a 0.25V interval.[t P =(t PHL +t PLH )/2].Using this data,plot ‘t P vs.V DD ’,and ‘Power vs.V DD ’.Specify AS,AD,PS,PD in your spice deck,and manually add C L =6.5fF.Set V SB =0Vfor this problem.b.For Vdd equal to 2.5V determine the maximum fan-out of identical inverters this gate candrive before its delay becomes larger than 2ns.c.Simulate the same circuit for a set of ‘pulse’inputs with rise and fall times of t in_rise,fall =1ns,2ns,5ns,10ns,20ns.For each input,measure (1)the rise and fall times t out_rise andV DD =2.5VV IN V OUTC L =C inv-gateL =L P =L N =0.25μmV SB-+(W p /W n =1.25/0.375)Figure 5.8CMOS inverter with capacitiveSection 5.1Exercises and Design Problems 187t out_fall of the inverter output,(2)the total energy lost E total ,and (3)the energy lost due to short circuit current E short .Using this data,prepare a plot of (1)(t out_rise +t out_fall )/2vs.t in_rise,fall ,(2)E total vs.t in_rise,fall ,(3)E short vs.t in_rise,fall and (4)E short /E total vs.t in_rise,fall.d.Provide simple explanations for:(i)Why the slope for (1)is less than 1?(ii)Why E short increases with t in_rise,fall ?(iii)Why E total increases with t in_rise,fall ?12.Consider the low swing driver of Figure 5.9:a.What is the voltage swing on the output node (V out )?Assume γ=0.b.Estimate (i)the energy drawn from the supply and (ii)energy dissipated for a 0V to 2.5V transition at the input.Assume that the rise and fall times at the input are 0.Repeat the analysis for a 2.5V to 0V transition at the input.pute t pLH (i.e.the time to transition from V OL to (V OH +V OL )/2).Assume the input rise time to be 0.V OL is the output voltage with the input at 0V and V OH is the output volt-age with the input at 2.5V .pute V OH taking into account body effect.Assume γ =0.5V 1/2for both NMOS and PMOS.13.Consider the following low swing driver consisting of NMOS devices M1and M2.Assumean NWELL implementation.Assume that the inputs IN and IN have a 0V to 2.5V swing andthat V IN =0V when V IN =2.5V and vice-versa.Also assume that there is no skew between INand IN (i.e.,the inverter delay to derive IN from IN is zero).a.What voltage is the bulk terminal of M2connected to?V in V out V DD =2.5V W L 3μm 0.25μm =p 2.5V0V C L =100fFW L 1.5μm 0.25μm=n Figure 5.9Low Swing DriverV LOW =0.5VOutM1ININ M225μm/0.25μm 25μm/0.25μmC L =1pFFigure 5.10Low Swing Driver188THE CMOS INVERTER Chapter 5b.What is the voltage swing on the output node as the inputs swing from 0V to 2.5V .Showthe low value and the high value.c.Assume that the inputs IN and IN have zero rise and fall times.Assume a zero skewbetween IN and IN.Determine the low to high propagation delay for charging the outputnode measured from the the 50%point of the input to the 50%point of the output.Assumethat the total load capacitance is 1pF,including the transistor parasitics.d.Assume that,instead of the 1pF load,the low swing driver drives a non-linear capacitor,whose capacitance vs.voltage is plotted pute the energy drawn from the lowsupply for charging up the load capacitor.Ignore the parasitic capacitance of the driver cir-cuit itself.14.The inverter below operates with V DD =0.4V and is composed of |V t |=0.5V devices.Thedevices have identical I 0and n.a.Calculate the switching threshold (V M )of this inverter.b.Calculate V IL and V IH of the inverter.15.Sizing a chain of inverters.a.In order to drive a large capacitance (C L =20pF)from a minimum size gate (with inputcapacitance C i =10fF),you decide to introduce a two-staged buffer as shown in Figure5.12.Assume that the propagation delay of a minimum size inverter is 70ps.Also assumeV DD =0.4VV IN V OUTFigure 5.11Inverter in Weak Inversion RegimeSection 5.1Exercises and Design Problems 189that the input capacitance of a gate is proportional to its size.Determine the sizing of thetwo additional buffer stages that will minimize the propagation delay.b.If you could add any number of stages to achieve the minimum delay,how many stages would you insert?What is the propagation delay in this case?c.Describe the advantages and disadvantages of the methods shown in (a)and (b).d.Determine a closed form expression for the power consumption in the circuit.Consider only gate capacitances in your analysis.What is the power consumption for a supply volt-age of 2.5V and an activity factor of 1?16.[M,None,3.3.5]Consider scaling a CMOS technology by S >1.In order to maintain compat-ibility with existing system components,you decide to use constant voltage scaling.a.In traditional constant voltage scaling,transistor widths scale inversely with S,W ∝1/S.To avoid the power increases associated with constant voltage scaling,however,youdecide to change the scaling factor for W .What should this new scaling factor be to main-tain approximately constant power.Assume long-channel devices (i.e.,neglect velocitysaturation).b.How does delay scale under this new methodology?c.Assuming short-channel devices (i.e.,velocity saturation),how would transistor widthshave to scale to maintain the constant power requirement?1InAdded Buffer StageOUTC L =20pF C i =10fF‘1’is the minimum size inverter.??Figure 5.12Buffer insertion for driving large loads.190THE CMOS INVERTER Chapter5DESIGN PROBLEMUsing the0.25μm CMOS introduced in Chapter2,design a static CMOSinverter that meets the following requirements:1.Matched pull-up and pull-down times(i.e.,t pHL=t pLH).2.t p=5nsec(±0.1nsec).The load capacitance connected to the output is equal to4pF.Notice that thiscapacitance is substantially larger than the internal capacitances of the gate.Determine the W and L of the transistors.To reduce the parasitics,useminimal lengths(L=0.25μm)for all transistors.Verify and optimize the designusing SPICE after proposing a first design using manual -pute also the energy consumed per transition.If you have a layout editor(suchas MAGIC)available,perform the physical design,extract the real circuitparameters,and compare the simulated results with the ones obtained earlier.。
数字集成电路 电路系统与设计 chapter1

Digital Cellular Market (Phones Shipped)
Power Management
1996 1997 1998 1999 2000
Units
48M 86M 162M 260M 435M
Analog Baseband
Digital Baseband (DSP + MCU)
8086
Source: Intel
1 Billion Transistors
Pentium® III Pentium® II Pentium® Pro Pentium®
i486 i386 80286
1 1975 1980 1985 1990 1995 2000 2005 2010
Projected
EE141 © Digital Integrated
Circuits2nd
Courtesy, Intel
Introduction
Power Dissipation
100 P6 Pentium ® proc 10 8086 286 1 8085 8080 486 386 Power (Watts)
8008 4004
0.1 1971 1974 1978 Year 1985 1992 2000
Circuits2nd
Courtesy, Intel
Introduction
14
Moore’s law in Microprocessors
1000 Transistors (MT) 100 10 1 0.1 8086 8080 8008 4004 1980 386 286 486
2X growth in 1.96 years!
数字逻辑与数字集成电路第2版第1章第2章01

a
b
c
t pLH
t pHL
典型的组合逻辑电路
(1)门电路
(Gates)
(2)译码电路 (Decoders)
编码电路 (Encoders)
(3)数据选择电路 (Multiplexer)(多路开关)
或数据选择器 (Data Selector)
(4) 加法器
(Adders)
算术逻辑单元 ( Arithmetic Logic Units )
与非门工作原理:(输 “H”
入为高)
“H” “L”
VA=VB=”H”=3.6V
IR1全部流向T2基极
输入漏电流IIH,从多发射极流入
T2 , T5饱和, T2基极的电压为1.4v, T2发射极(T5基极)的电压
为0.7V。由于T5饱和,所以:
图
输出电压: VoL =Vces5=0.1~0.3V =”L” 输出电流 IoL:从外电路流向T5
开关特性
在T2由饱和向截止转换时,VC2升高,使T3、 T4同时导通,“1”驱动级给尚未脱离饱和的 T5提供很大集流,从而使T5迅速脱离饱和。 在T5脱离饱和时,VC2抬高,Ib5随之减少, 这时T5吸收不了由T3,T4流来的电流,它们大 部分流向输出负载电容,使它迅速充电,加 快输出电压上升
R3为T5基区电荷的逸散提供了通路,使T5截 止过程加快
半导体制造工艺的发展带动了集成电路的更新换代。 VLSI时代存储器件制造工艺带动了整个微处理器的更
新换代。 摩尔定律:每18个月集成度翻一翻。 集成电路内部的连线宽度是主要的指标: 0.8 m, 0.35 m, 0.25m, 0.18m,0.13 m …….
集成电路发展历史(续)
(1) Small Scale IC (SSI)
数字集成电路——电路、系统与设计

数字集成电路——电路、系统与设计目录第一部分基本单元第1章引论1.1 历史回顾1.2 数字集成电路设计中的问题1.3 数字设计的质量评价1.4 小结1.5 进一步探讨第2章制造工艺2.1 引言2.2 CMOS集成电路的制造2.3 设计规则——设计者和工艺工程师之间的桥梁2.4 集成电路封装2.5 综述:工艺技术的发展趋势2.6 小结2.7 进一步探讨设计方法插入说明A——IC版图第3章器件3.1 引言3.2 二极管3.3 MOS(FET)晶体管3.4 关于工艺偏差3.5 综述:工艺尺寸缩小3.6 小结3.7 进一步探讨设计方法插入说明B——电路模拟第4章导线4.1 引言4.2 简介4.3 互连参数——电容、电阻和电感4.4 导线模型4.5 导线的SPICE模型4.6 小结4.7 进一步探讨第二部分电路设计第5章CMOS反相器5.1 引言5.2 静态CMOS反相器——直观综述5.3 CMOS反相器稳定性的评估——静态特性5.4 CMOS反相器的性能——动态特性5.5 功耗、能量和能量延时5.6 综述:工艺尺寸缩小及其对反相器衡量指标的影响5.7 小结本文由整理提供5.8 进一步探讨第6章CMOS组合逻辑门的设计6.1 引言6.2 静态CMOS设计6.3 动态CMOS设计6.4 设计综述6.5 小结6.6 进一步探讨设计方法插入说明C——如何模拟复杂的逻辑电路设计方法插入说明D——复合门的版图技术第7章时序逻辑电路设计7.1 引言7.2 静态锁存器和寄存器7.3 动态锁存器和寄存器7.4 其他寄存器类型7.5 流水线:优化时序电路的一种方法7.6 非双稳时序电路7.7 综述:时钟策略的选择7.8 小结7.9 进一步探讨第三部分系统设计第8章数字IC的实现策略8.1 引言8.2 从定制到半定制以及结构化阵列的设计方法8.3 定制电路设计8.4 以单元为基础的设计方法8.5 以阵列为基础的实现方法8.6 综述:未来的实现平台8.7 小结8.8 进一步探讨设计方法插入说明E——逻辑单元和时序单元的特性描述设计方法插入说明F——设计综合第9章互连问题9.1 引言9.2 电容寄生效应9.3 电阻寄生效应9.4 电感寄生效应9.5 高级互连技术9.6 综述:片上网络9.7 小结9.8 进一步探讨第10章数字电路中的时序问题10.1 引言10.2 数字系统的时序分类本文由整理提供10.3 同步设计——一个深入的考察10.4 自定时电路设计10.5 同步器和判断器10.6 采用锁相环进行时钟综合和同步10.7 综述:未来方向和展望10.8 小结10.9 进一步探讨设计方法插入说明G——设计验证第11章设计运算功能块11.1 引言11.2 数字处理器结构中的数据通路11.3 加法器11.4 乘法器11.5 移位器11.6 其他运算器11.7 数据通路结构中对功耗和速度的综合考虑11.8 综述:设计中的综合考虑11.9 小结11.10进一步探讨第12章存储器和阵列结构设计12.1 引言12.2 存储器内核12.3 存储器外围电路12.4 存储器的可靠性及成品率12.5 存储器中的功耗12.6 存储器设计的实例研究12.7 综述:半导体存储器的发展趋势与进展12.8 小结12.9 进一步探讨设计方法插入说明H——制造电路的验证和测试本文由整理提供。
数字集成电路--电路、系统与设计(第二版)课后练习题 第六章 CMOS组合逻辑门的设计-Chapter 6 Designing
4
Chapter 6 Problem Set
VDD F G
A B
A
A B
A
Figure 6.6 Two-input complex logic gate.
11.
Design and simulate a circuit that generates an optimal differential signal as shown in Figure 6.7. Make sure the rise and fall times are equal.
2
VDD E 6 A A 6 B 6 C 6 D 6 F A B C D 4 4 4 4 E 1 A B C D E 4 4 4 4 E 1 6 F 6 B 6 C 6 D
Chapter 6 Problem SetVDD 6Circ来自it ACircuit B
Figure 6.2 Two static CMOS gates.
Digital Integrated Circuits - 2nd Ed
3
2.5 V
PMOS
M2 W/L = 0.5μm/0.25μm Vout Vin M1 W/L = 4μm/0.25μm NMOS Figure 6.4 Pseudo-NMOS inverter.
a. What is the output voltage if only one input is high? If all four inputs are high? b. What is the average static power consumption if, at any time, each input turns on with an (independent) probability of 0.5? 0.1? c. Compare your analytically obtained results to a SPICE simulation.
数字集成电路电路系统与设计第二版教学设计

数字集成电路电路系统与设计第二版教学设计一、教学目标本次教学旨在让学生了解数字集成电路的基础知识、电路系统的设计方法以及数字系统的逻辑设计方法。
具体目标如下:1.理解数字集成电路的分类和特点;2.掌握数字电路的基础知识,如布尔代数、真值表等;3.熟悉数字电路的常用逻辑门电路,并能够根据要求进行设计;4.掌握数字系统的逻辑设计方法,包括组合逻辑和时序逻辑;5.进一步了解数字系统的设计流程和仿真方法;6.培养学生的实验思维和实验能力,使其能够进行数字系统的设计和实现。
二、教学内容2.1 数字集成电路的基础知识1.数字集成电路的定义和分类;2.数字集成电路的特点和应用。
2.2 数字电路的基础知识1.布尔代数和布尔运算;2.真值表和逻辑表达式;3.逻辑门电路,包括与门、或门、非门、与非门、或非门、异或门、同或门等。
2.3 数字系统的逻辑设计方法1.组合逻辑,包括编码器、译码器、选择器、加法器、减法器等;2.时序逻辑,包括时钟信号的产生和应用、触发器和寄存器的设计等。
2.4 数字系统的设计流程和仿真方法1.数字系统的设计流程,包括需求分析、设计规划、电路设计、仿真和调试等;2.数字系统的仿真方法,包括计算机仿真、逻辑仿真和时序仿真等。
2.5 数字系统的实验设计1.了解数字系统的实验室器材和实验平台;2.完成数字系统实验的设计、仿真和调试。
三、教学方法本次教学采用理论与实践相结合的方法,通过课堂讲授、实验设计和课后作业等方式,提高学生的实践能力和解决实际问题的能力。
具体方法如下:1.采用多媒体课件,结合教材,进行知识点的讲解;2.提供实验器材和数字系统仿真软件,让学生进行数字系统的设计、仿真和调试;3.组织小组讨论,进行案例分析和问题解决;4.布置设计任务,让学生独立完成数字系统设计和实现,并提交报告;5.课后作业,包括习题和实验报告等,巩固学生的知识点和实践能力。
四、教学评价本次教学采用成果评价和过程评价相结合的方法。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
INTRODUCTION
Chapter 1
1.1
A Historical Perspective The concept of digital data manipulation has made a dramatic impact on our society. One has long grown accustomed to the idea of digital computers. Evolving steadily from mainframe and minicomputers, personal and laptop computers have proliferated into daily life. More significant, however, is a continuous trend towards digital solutions in all other areas of electronics. Instrumentation was one of the first noncomputing domains where the potential benefits of digital data manipulation over analog processing were recognized. Other areas such as control were soon to follow. Only recently have we witnessed the conversion of telecommunications and consumer electronics towards the digital format. Increasingly, telephone data is transmitted and processed digitally over both wired and wireless networks. The compact disk has revolutionized the audio world, and digital video is following in its footsteps. The idea of implementing computational engines using an encoded data format is by no means an idea of our times. In the early nineteenth century, Babbage envisioned largescale mechanical computing devices, called Difference Engines [Swade93]. Although these engines use the decimal number system rather than the binary representation now common in modern electronics, the underlying concepts are very similar. The Analytical Engine, developed in 1834, was perceived as a general-purpose computing machine, with features strikingly close to modern computers. Besides executing the basic repertoire of operations (addition, subtraction, multiplication, and division) in arbitrary sequences, the machine operated in a two-cycle sequence, called “store” and “mill” (execute), similar to current computers. It even used pipelining to speed up the execution of the addition operation! Unfortunately, the complexity and the cost of the designs made the concept impractical. For instance, the design of Difference Engine I (part of which is shown in Figure 1.1) required 25,000 mechanical parts at a total cost of £17,470 (in 1834!).
Section 1.1
A Historical Perspective
11
The electrical solution turned out to be more cost effective. Early digital electronics systems were based on magnetically controlled switches (or relays). They were mainly used in the implementation of very simple logic networks. Examples of such are train safety systems, where they are still being used at present. The age of digital electronic computing only started in full with the introduction of the vacuum tube. While originally used almost exclusively for analog processing, it was realized early on that the vacuum tube was useful for digital computations as well. Soon complete computers were realized. The era of the vacuum tube based computer culminated in the design of machines such as the ENIAC (intended for computing artillery firing tables) and the UNIVAC I (the first successful commercial computer). To get an idea about integration density, the ENIAC was 80 feet long, 8.5 feet high and several feet wide and incorporated 18,000 vacuum tubes. It became rapidly clear, however, that this design technology had reached its limits. Reliability problems and excessive power consumption made the implementation of larger engines economically and practically infeasible. All changed with the invention of the transistor at Bell Telephone Laboratories in 1947 [Bardeen48], followed by the introduction of the bipolar transistor by Schockley in 1949 [Schockley49]1. It took till 1956 before this led to the first bipolar digital logic gate, introduced by Harris [Harris56], and even more time before this translated into a set of integrated-circuit commercial logic gates, called the Fairchild Micrologic family [Norman60]. The first truly successful IC logic family, TTL (Transistor-Transistor Logic) was pioneered in 1962 [Beeson62]. Other logic families were devised with higher performance in mind. Examples of these are the current switching circuits that produced the first subnanosecond digital gates and culminated in the ECL (Emitter-Coupled Logic) family [Masaki74]. TTL had the advantage, however, of offering a higher integration density and was the basis of the first integrated circuit revolution. In fact, the manufacturing of TTL components is what spear-headed the first large semiconductor companies such as Fairchild, National, and Texas Instruments. The family was so successful that it composed the largest fraction of the digital semiconductor market until the 1980s. Ultimately, bipolar digital logic lost the battle for hegemony in the digital design world for exactly the reasons that haunted the vacuum tube approach: the large power consumption per gate puts an upper limit on the number of gates that can be reliably integrated on a single die, package, housing, or box. Although attempts were made to develop high integration density, low-power bipolar families (such as I2L—Integrated Injection Logic [Hart72]), the torch was gradually passed to the MOS digital integrated circuit approach. The basic principle behind the MOSFET transistor (originally called IGFET) was proposed in a patent by J. Lilienfeld (Canada) as early as 1925, and, independently, by O. Heil in England in 1935. Insufficient knowledge of the materials and gate stability problems, however, delayed the practical usability of the device for a long time. Once these were solved, MOS digital integrated circuits started to take off in full in the early 1970s. Remarkably, the first MOS logic gates introduced were of the CMOS variety [Wanlass63], and this trend continued till the late 1960s. The complexity of the manufacturing process delayed the full exploitation of these devices for two more decades. Instead,
