环境化学_多介质环境模型
持久性有机污染物环境多介质空间分异模型研究进展_刘世杰

2011年 第6卷第2期,129-137生态毒理学报A si an Journal o f Ecotox icologyV o.l 6,2011N o .2,129-137收稿日期:2010-12-01 录用日期:2010-12-30基金项目:国家自然科学基金面上项目(41071355);国家重点基础研究发展规划(973)项目(2007CB 407307);中瑞合作项目(G J H Z0910) 作者简介:刘世杰(1986-),男,硕士研究生,主要从事环境管理方面的研究工作,E-m ai:l li us j 04@g m ai.l com;*通讯作者(Correspond i ngauthor),E-m ai:l yll u@rcees .ac .cn持久性有机污染物环境多介质空间分异模型研究进展刘世杰1,2,吕永龙1,*,史雅娟11.中国科学院生态环境研究中心城市与区域生态国家重点实验室,北京1000852.中国科学院研究生院,北京100049摘要:环境多介质空间分异模型能够对持久性有机污染物(POP s)在环境多个介质中空间尺度上的迁移转化和分配过程进行准确、细致和接近真实的描述,是进行PO Ps 的环境多介质归趋模拟和环境风险评价的重要工具。
将环境多介质空间分异模型分为环境多介质质量平衡空间区划模型和大气化学传输模型,对目前几种常用的环境多介质空间分异模型GLO B O-PO P 、C lM i oChe m 、BETR 、I M PACT 2002、G-C IE M S 、M SCE -POP 和DE HM-POP 模型的基本情况进行了介绍,并分析了这些模型近十年来在国内外的开发和应用情况。
对当前国内外环境多介质空间分异模型研究中存在的不足进行了分析,指出监测手段的落后、基础环境参数的不足和实验数据的稀缺是制约国内此类模型发展的主要因素,并对环境多介质空间分异模型的开发和应用前景进行了展望。
《环境化学》课程教学大纲

《环境化学》课程教学大纲一、基本信息课程性质:专业选修课学分:2学分学时:32学时(讲授24学时、实验8学时)开课学期:第三学期开课院系:环境工程与化学学院适用专业:环境工程先修课程:有机化学、无机及分析化学、物理化学、仪器分析、二、课程简介《环境化学》是环境工程专业的选修课,通过该课程的学习,使学生掌握污染物在大气、水体、土壤的迁移原理,以及生物富集、降解作用和环境修复的主要理论基础,理解污染特征与转化规律,树立环境保护意识和生态文明建设理念,为进一步学习专业课程,从事环境相关工作奠定必要的理论基础。
三、课程教学目标(一)教学任务本课程的主要任务是通过课堂教学使学生能够掌握环境化学中大气、水体、土壤和生物体的本质特征,相关元素和污染物在环境中迁移、转化的基本原理和途径。
了解目前环境修复的基础理论和前沿技术,并能够初步分析复杂的环境工程问题,提出具体恰当、合理的处理方案。
(二)课程目标1. 课程目标(1)掌握环境化学中大气、水体、土壤和生物体的本质属性和特征,污染物在其中的迁移、转化的基本概念、途径和原理(支撑毕业要求2.1)。
(2)了解环境化学中环境修复的相关技术、原理和前沿进展,能够通过查阅文献资料并运用相关知识和技术,识别、判断、评价复杂的环境污染问题,并能提出合理的处理方案(支撑毕业要求2.1)。
2. 课程目标对毕业要求的支撑矩阵表1 课程目标对毕业要求的支撑矩阵(三)思政元素根据本课程性质和特点,在教学环节中对生态文明建设、环境保护、可持续发展思政元素进行落实。
四、教学内容及要求表2 教学内容、要求与达成五、课内实验名称及基本要求表3 实验内容、要求与达成六、课程考核方式与评分标准(一)考核方式表4 课程目标的考核方式注:考核内容体现对课程思政元素的理解要求。
(二)总成绩构成本课程考核由形成性评价(40%)和结果性评价(60%)构成,其中形成性评价包括课堂讨论、习题作业和实验三个部分,分别占总成绩的5%、10%和20%,结果性评价以期末考试为主。
环境多介质归趋模型简介

5/30/2020
9
3. 非稳态方程
前两类质量平衡方程是简单的代数等式,而在 非稳态条件下的质量平衡方程是微分方程。最简单 的方法是列出如下方程:
dC/dt=总的输入速率一总的输出速率 式中,dC是浓度增量, dt是时间增量,输入、
输出的速率单位为mol·h-1或g·h-1。
5/30/2020
10
一般而言,真实的环境系统和我们模拟的环 境系统之间存在一定的差距,在构建模型时, 需要用一系列的假设来简化真实的环境系统, 比如:
环境体系由多个环境主相和若干子相组成; 任一时刻,每个环境主相的污染物呈均匀分 布,各子相之间的逸度关系符合平衡稳态,即 逸度值在同一时刻处处相等; 环境主相之间处于不平衡动态。
二、逸度
逸度(fugacity)是一热力学量,它表示 物质脱离某一相的倾向性的大小,其单位 为压力单位(Pa)。作为判别各相间平衡标准 的一种简便方法,于1901年G.N.Lewis 提出。用逸度代替浓度,应用到多介质环 境模型中,简化了模型的计算,因而得到 了广泛应用。
5/30/2020
11
在多介质环境模型中,如果污染物在研究系统各
5/30/2020
16
例如,对于一个由大气、地表水、沉积物 和土壤四个环境主相组成的环境系统。每 个环境主相下面又分为若干个环境子相。
大气由气体和颗粒物两个子相组成,地表 水由悬浮颗粒物和水两个子相组成,沉积 物和土壤都包含固相子相、气相子相和液 相子相。
5/30/2020
17
(二)污染物的环境迁移过程
大量研究表明,多介质环境模型是研究生态环
境中污染物,特别是有机污染物多介质环境行为的 有效手段。
5/30/2020
环境化学中常用模型介绍
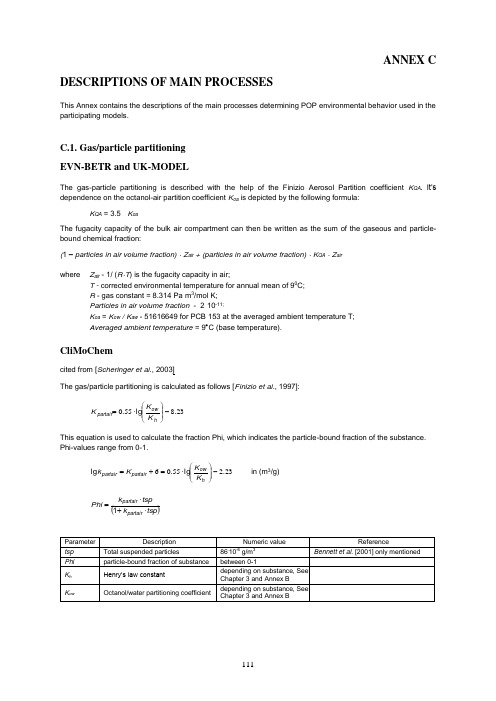
ANNEX CDESCRIPTIONS OF MAIN PROCESSESThis Annex contains the descriptions of the main processes determining POP environmental behavior used in the participating models.C.1. Gas/particle partitioning EVN-BETR and UK-MODELThe gas-particle partitioning is described with the help of the Finizio Aerosol Partition coefficient K QA . It’s dependence on the octanol-air partition coefficient K oa is depicted by the following formula:K QA = 3.5 · K oaThe fugacity capacity of the bulk air compartment can then be written as the sum of the gaseous and particle-bound chemical fraction:(1 – particles in air volume fraction) · Z air + (particles in air volume fraction) · K QA · Z airwhere Z air - 1/ (R·T ) is the fugacity capacity in air;T - corrected environmental temperature for annual mean of 90C;R - gas constant = 8.314 Pa·m 3/mol K;Particles in air volume fraction - 2·10-11;K oa = K ow / K aw - 51616649 for PCB 153 at the averaged ambient temperature T; Averaged ambient temperature = 9︒C (base temperature).CliMoChemcited from [Scheringer et al ., 2003]The gas/particle partitioning is calculated as follows [Finizio et al ., 1997]:238550.lg .-⎪⎪⎭⎫⎝⎛⋅=howpartair KK K This equation is used to calculate the fraction Phi, which indicates the particle-bound fraction of the substance. Phi-values range from 0-1.2325506.lg .lg -⎪⎪⎭⎫⎝⎛⋅=+=howpartair partair KK K k in (m 3/g) ()tsp k tspk Phi partair partair ⋅+⋅=1G-CIEMSWhen K oa is not available as input:K qa = 6 · 106/P ls ,where K qa is dimensionless particle/gas partition coefficient and P ls is liquid vapour pressure. Final partitioning iscalculated with TSP and density of aerosol particles in fugacity format. Vapour pressure is temperature corrected when the temperature is different from 25 ︒C. When K oa is available as input:K qa = y · K oa / (ρ/1000),where, K qa is dimensionless particle/gas partition coefficient, y is organic matter mass fraction, and ρis thedensity of aerosol particles.(Note: G-CIEMS model can calculate V/P partitioning from only molecular weight (for preliminary assessment purpose) or from K oa . Two output 1 and 2 is presented in Chapter 4 as G-CIEMS-1 and G-SIEMS-2).DEHM-POPThe gas-particle partitioning is calculated using the absorption model:,)(1+=φTSP K TSP K p pwhere φ is the fraction of compound sorbed to particles, K p is gas-particle partitioning coefficient, and TSP is the total suspended particulate matter [e.g. Falconer and Harner , 2000]. K p is calculated using the K oa approach:log ,91.11log log -+=om oa r p f K m Kwhere m r is a constant expected to have a value close to +1 for equilibrium partitioning, K oa is the octanol-air partitioning coefficient, f om is the fraction of organic matter in the particles, and 11.91 is a constant determined by the intercept br = log f om – 11.91 [Finizio et al ., 1997, Falconer and Harner , 2000]. The temperature dependent K oa is calculated using the expression:)),11(ex p()()(TT R U T K T K ref oa ref oa oa -∆= where ∆U oa is the internal energy of phase transfer, R is the universal gas constant, T is the temperature and K oa (T ref ) is the value of K oa at the reference temperature T ref [Beyer et al., 2002].SimpleBoxcited from [Brandes et al ., 1996]Air-aerosol partition coefficients are usually not known. However, some information is frequently available on the fraction of the chemical that occurs in association with the aerosol phase. SimpleBox uses this information for the computations. A value for the fraction of the chemical that is associated with the aerosol phase, FRass aerosol , can be entered directly, or estimated on the basis of the chemical's vapor pressure, according to Junge [1977]. In this equation, the sub-cooled liquid vapour pressure should be used. For solids, a correction is applied according to Mackay [1991]:If MELTINGPOINT < TEMPERATURE [S] (substance is liquid):θθCONST.+T URE VAPORPRESS CONST.= FRass S aerosol )(][ If MELTINGPOINT > TEMPERATURE [S] (substance is solid):θθ-CONST.+eT URE VAPORPRESS CONST.= FRass S E TE M P E RA TUR NTM E LTINGP OI S aerosol ).(.][][).(1796withFRassa erosol[S] - fraction of the chemical in air that is associated with aerosol particles at scale S [-] (A); VAPOR PRESSURE(T) - vapor pressure of the chemical at temperature T at scale S [Pa] (A);MELTINGPOINT - melting point of the chemical [K] (A); CONST- constant [Pa·m] (C);θ- surface area of aerosol phase [m aerosol 2/m air 3] (C);TEMPERATURE [S ]- temperature at the air-water interface at scale S [K] (A).with the product CONST ⋅ θ set equal to 10-4Pa.CAM/POPsIn the CAM/POPs model, the process of POP partitioning between the gas and particulate phases in atmosphereis based on Junge-Pankow adsorption model [Junge , 1977; Pankow , 1987] POP fraction Φ adsorbed on the atmospheric aerosol particles is given by:Θ⋅+Θ⋅=Φc P c Lwhere, Φ - fraction of POPs adsorbed on aerosol particles;Θ - aerosol surface area available for adsorption, m 2aerosol/m 3air; P 0L - liquid-phase saturation vapour pressure of pure compound, Pa;c - parameter that depends on the thermodynamics of the adsorption process and surface properties of the aerosol (Pa · cm).Junge’s proposed value of the parameter c is 17.2 Pa · cm [P ankow , 1987; Falconer et al., 1994; Bidleman et al.,1998].The liquid vapour pressure, P 0L , are derived from:b TmP L +=010log , where the slope (m ) and the intercept (b ) are estimated to calculate liquid vapour pressure of POPs withchanging air temperature [Falconer et al., 1995; Harner et al., 1996]. Temperature dependence of P 0L for each congener can be seen in Table C.1.Table C.1. Liquid vapour pressure of PCBs as a function of air temperatureAerosol surface area, Θ, is calculated by multiplying aerosol number density by its wet surface area.MSCE-POPIn the current model version (MSCE-POP 1) the characterization of POP partitioning between the gas and particulate phase of a pollutant is performed using subcooled liquid vapour pressure p ol (Pa). According to the Junge-Pankow adsorption model [Junge , 1977; Pankow , 1987] POP fraction ϕ adsorbed on the atmospheric aerosol particles equals to: θϕ⋅+⋅=c p c olwherec is the constant depending on thermodynamic parameters of adsorption process and on properties of aerosol particle surface. It assumed c = 0.17 Pa·m [Junge , 1977] for background aerosol;θ is the specific surface of aerosol particles, m 2/m 3. Assumed θ = 1.5·10-4 [Whitby , 1978].The temperature dependence of p ol (Pa) is parameterized in the model by:⎪⎭⎫ ⎝⎛--⋅=0110T T a olol P ep p ,where T 0 = 283.15 K is the reference temperature, T (K) is the ambient temperature, p 0ol is p ol value at referencetemperature, and a P is the coefficient of temperature dependence. The values of p 0oland a P for considered PCB congeners used in the model are presented in Table C.2.Table C.2. Coefficients of p ol temperature dependence for three PCB congeners used in MSCE-POP modelAt present the work on modification of the description of gas aerosol partitioning within MSCE-POP model is ongoing. The approach using the octanol-air partitioning coefficient absorption model presented in [Falconer and Harner, 2000] is tested. Under this approach POP fraction ϕ adsorbed on the atmospheric aerosol particles is calculated as:TSPK TSP K p p ⋅+⋅=ϕ1where K p is the particle-gas partitioning coefficient and TSP is the total suspended particle concentration (μg ⋅m -3). The constant K p is calculated for PCBs via K oa by the following regression equations [Falconer and Harner, 2000]:log K p = log K oa + log f om – 11.91,(experimental version - MSCE-POP 2)where K oa is the octanol/air partitioning coefficient and f om is the fraction of organic matter in the atmospheric aerosol involved in partitioning.The temperature dependence of K oa is parameterized in the model by: ⎪⎭⎫ ⎝⎛--⋅=0110T T a oaoa K eK K ,where Koa is K oa value at reference temperature, and a K is the coefficient of temperature dependence.The values of Koa and a for considered PCB congeners used in the model are presented in Table C.3.Table C.3. Coefficients of K oa temperature dependence for three PCB congeners used in MSCE-POP modelEVN-BETR and UK-MODELThe intermedia transport of chemicals is described using D-values (mol/Pa·h), which represent how fast advective and reactive/degradation processes are occurring. In the case of the air to surface exchange, the D-value defining dry particle deposition is:Dair-surface = Surface Area · Particles in air volume fraction · V q · Z air · K QAKnowing these values can help calculate the flux of a chemical entering a region and, thus, it’s amount in thecompartment under study.Surface area - compartment specific;Particles in air volume fraction - 2 10-11; Vq - dry deposition velocity = 10.8 m/h.CliMoChemcited from [Scheringer et al ., 2003]Dry deposition to baresoil a , water, vegetation-covered soil bgas gas i drygas C V A v Phi dtdC ⋅⎪⎪⎭⎫⎝⎛⎪⎪⎭⎫ ⎝⎛⋅-=aIf the year consists of exactly four seasons with varying temperatures, v dry for deposition to baresoil is changing taking into account that in the cold season the atmosphere is more stable and the deposition rate therefore is smaller. The spring and fall values are interpolations between the summer and winter values. v dry changes as follows [Wania and McLachlan , 2001]: bIf the year consists of exactly four seasons with varying temperatures, v dry for deposition to vegetation-covered soil is changing taking into account that in the cold season the atmosphere is more stable and the deposition rate therefore is smaller. The spring and fall values are interpolations between the summer and winter values. v dry changes as follows [Wania and McLachlan , 2001]: The vegetation cover consists of three types: Grass, Coniferous Forest and Deciduous Forest. The variables VegGrass, VegCon and VegDec describe the fraction of the vegetation-covered soil occupied by the different cover types. Their numeric value is between 0-1 and depends on the climatic zone.Dry deposition to vegetationgas gas veg gas C V A vdry Phi dtdC ⋅⎪⎪⎭⎫⎝⎛⎪⎪⎭⎫⎝⎛⋅-=* - the model contains three types of vegetation. For each type, the deposition rate (v dry ) is different (see table below). Depending on the composition of a climatic zone, v dry is calculated as follows:con i dec i grass i i vdry n fractionco vdry c fractionde vdry ass fractiongr vdry ⋅+⋅+⋅=Because of increased stability of the atmosphere in the spring, fall and winterseason, the deposition rates vdrygrass, vdrydec and vdrycon are divided by 3 for the winterseason and by 2 for the spring and fall seasons (given that the year consists of exactly four seasons with varying temperature [Wania and McLachlan , 2001]).G-CIEMSF = v Dep · (TSP/ρ) · C particleWhere F is mass flux of compound for this chemical, v Dep is dry deposition velocity of particles, TSP is particulateconcentration of weight/volume dimension , ρ is density of aerosol particles, C particle is compound volumetric concentration in particles. Same value is used on all land and water surfaces.DEHM-POPThe dry deposition of particulate phase is calculated as a flux given by the atmospheric concentration times a deposition velocity [Christensen , 1997]. The deposition velocity is highly dependent on the meteorological conditions and the surface properties. The size of the particles is assumed to be 1μm [Christensen , 1997]. For unstable conditions in the atmosphere (when L < 0, i.e. at day time with clear sky), the deposition velocity is calculated using:),)300(1()3/2(*La u v d -+=where u* is the surface friction velocity, a is a constant depending on the surface properties, and L is the Monin-Obukhov length.For stable conditions in the atmosphere (when L > 0, i.e. at night time with clear sky), the deposition velocity iscalculated using:.*au v d =The surface friction velocity is calculated using:,*)10ln(*35.0*zUu =where U is the wind speed and z is the roughness length which depends on the properties of the surface, and varies seasonally.The Monin-Obukhov length is calculated using:,)(.)(*ρ-⋅⋅⋅=p dc H g Tu L 3503 where T is the temperature, g is the gravitational constant (g = 9.82), H d is the heat flux, c p is the specific heat at constant pressure, and ρ is the air density. L is positive (stable atmosphere) when the heat flux is negative (night time clear sky) and negative (unstable atmosphere) when the heat flux is positive (day time clear sky).SimpleBoxcited from [Brandes et al ., 1996]Value for the deposition mass transfer coefficients DRYDEP aerosol may be obtained by means of:FRass .RATE AEROSOLDEP = DRYDEP S aerosol S S aerosol ][][][with DRYDEP aerosol [S ]: mass transfer coefficient for dry deposition of aerosol-associated chemical at scale S[m air /s] (D);AEROSOLDEPRATE [S ] - deposition velocity of the aerosol particles at scale S with which the chemical is associated [m/s] (A);FRassaerosol [S ]: fraction of the chemical in air that is associated with aerosol particles at scale S [-] (A). Deposition mass flows of the chemical depend on the rate of dry aerosol deposition. Deposition velocities of aerosols vary greatly with the size of the particles. As chemicals may be associated with particles of a specific size, the deposition velocities depend also on the chemical. The values given are typical values, to be used as a starting point:scm = RATE AEROSOLDEP S /1.0][with AEROSOLDEPRATE [S ]:deposition velocity of the aerosol particles with which the chemical isassociated at scale S [m/s] (A)CAM/POPsRemoval of POPs particles is coupled with the dry deposition of aerosols in the CAM model. The dry deposition flux can be written as:,p d d C v F ⋅-=where V d is the deposition velocity and C p is the particle concentration [G ong et al. 2003]. Dry deposition flux of POPs gas is written as:,g d d C v F ⋅-=where the dry deposition velocity of gas, V d , is calculated in CAM model [G ong et al. 2003].MSCE-POPDry deposition to grass and bare soilAccording to model of [Sehmel , 1980], deposition flux over grass is calculated as (),*so il C soil soil p z B u A C F 02+⋅=whereas above *u , is the friction velocity; z 0 is the surface roughness, mm;A soil = 0.02,B soil = 0.01,C soil = 0.33.Dry deposition to forestAccording to model of [Ruijgrok et al., 1997], deposition flux over forest is calculated ashp u u EC F 2*⋅=,where u h is the wind speed at forest height h = z b ;*u is the friction velocity, m/s;()()()20/80ex p 1*-+=RH u E γαβ, α = 0.048, β = 0.3 and γ = 0.25, RH =80.Dry deposition to seawaterAccording to model of [Lindfors et al., 1991], deposition flux over seawater is calculated as: )(*sea sea p B u A C F +⋅=2,where *u is the friction velocity, m/s;A sea = 0.15,B sea = 0.013Similar to DEHM-POP model values of deposition flux depend on meteorological conditions (friction velocity u *).Therefore, in the results of calculation experiments we present the range of obtained values of the flux together with its value at average environmental parameters.C.3. Wet depositionEVN-BETR and UK-MODELIn a similar way as for the dry particle deposition, wet scavenging is defined as the result of:D air-surface = Q · Surface Area · Particles in air fraction · K QA · U R · Z airIn the case of deposition in vegetation, the percentage of rain interception due to vegetation is taken into account. U R - rain rate = 8.84 x 10-5m/h; Q - Rain Scavenging ratio = 200000; Or Snow Scavenging Ratio = 1000000CliMoChemcited from [Scheringer et al ., 2003]Wet deposition from gaseous phase to baresoil and water()gas h gas i rain gas C K V A v Phi dtdC ⋅⎪⎪⎭⎫⎝⎛⋅⎪⎪⎭⎫ ⎝⎛⋅--=11 , 1991 Wet deposition from gaseous phase to vegetation-covered soil()()gas rt h gas vsoil rain gas C f K V A v Phi dtdC ⋅-⋅⎪⎪⎭⎫⎝⎛⋅⎪⎪⎭⎫ ⎝⎛⋅--=111 particle-bound fraction of the substance (see C.1.) *- for deciduous and coniferous forest, the f rf -value is 0.35 for the summer season, for grass, the f rf -value is 0.12 for the summer season. In the winter season, the value is at 10% of the summer season, in spring and fall season, the value is at the linear interpolation value between summer and winter season. Because the composition of the vegetation varies with the climatic zones, the contributions of grass, coniferous and deciduous forest to the overall f rf -value of a specific climate zone differ and are proportional to the fraction of the respective vegetation type in a climatic zone.Wet deposition from gaseous phase to vegetation()gas rt h gas vsoil rain gas C f K V A v Phi dtdC ⋅⋅⎪⎪⎭⎫⎝⎛⋅⎪⎪⎭⎫ ⎝⎛⋅--=11 * - for deciduous and coniferous forest, the f rf -value is 0.35 for the summer season, for grass, the f rf -value is 0.12 for the summer season. In the winter season, the value is at 10% of the summer season, in spring and fall season, the value is at the linear interpolation value between summer and winter season. Because the composition of the vegetation varies with the climatic zones, the contributions of grass, coniferous and deciduous forest to the overall f rf -value of a specific climate zone differ and are proportional to the fraction of the respective vegetation type in a climatic zone.Wet deposition from particulate phase to bare soil and watergas gas i ratiorain gas C V A scav v Phi dtdC ⋅⎪⎪⎭⎫⎝⎛⎪⎪⎭⎫ ⎝⎛⋅⋅-=Wet deposition from particulate phase to vegetation-covered soil()gas rt gas vsoil ratiorain gas C f V A scav v Phi dtdC ⋅-⋅⎪⎪⎭⎫⎝⎛⎪⎪⎭⎫⎝⎛⋅⋅-=1* - for deciduous and coniferous forest, the f rf -value is 0.35 for the summer season, for grass, the f rf -value is 0.12 for the summer season. In the winter season, the value is at 10% of the summer season, in spring and fall season, the value is at the linear interpolation value between summer and winter season. Because the composition of the vegetation varies with the climatic zones, the contributions of grass, coniferous and deciduous forest to the overall f rf -value of a specific climate zone differ and are proportional to the fraction of the respective vegetation type in a climatic zone.Wet deposition from particulate phase to vegetationgas rt gas vsoil ratiorain gas C f V A scav v Phi dtdC ⋅⋅⎪⎪⎭⎫⎝⎛⎪⎪⎭⎫⎝⎛⋅⋅-= *- for deciduous and coniferous forest, the f rf -value is 0.35 for the summer season, for grass, the f rf -value is 0.12 for the summer season. In the winter season, the value is at 10% of the summer season, in spring and fall season, the value is at the linear interpolation value between summer and winter season. Because the composition of the vegetation varies with the climatic zones, the contributions of grass, coniferous and deciduous forest to the overall f rf -value of a specific climate zone differ and are proportional to the fraction of the respective vegetation type in a climatic zone.G-CIEMSF = R rain · C air / H +(TSP/ρ) · Q · C particle ,where F is total mass flux by this process, R rain is rain rate, C air is gaseous concentration of chemical, H isHenry ’s law constant, TSP is particulate concentration, ρ is density of particles, Q is scavenging ratio of particles, and C particle is volumetric chemical concentration in particles. Same Q value is assumed over all land and water surfaces.DEHM-POPThe wet deposition is given as a flux calculated as the product between the air concentration and a scavenging ratio [Christensen , 1997]. Different scavenging ratios are used for in-cloud scavenging and below-cloud scavenging. It is assumed that air pollution is scavenged more efficient in the clouds than below the clouds. The below cloud scavenging rate at a given height σ is given by:,)()(wa bc H P W ρσΛ=σwhere Λbc is the below cloud scavenging coefficient, P a is the total precipitation at the level, H is an effective thickness for scavenging (H = 1000 m), and ρw is the density of water. The in cloud scavenging rate at a given height σ is given by:,)()(wc H P W ρσΛ=σ where Λc is the below cloud scavenging coefficient, P a is the is the total precipitation created inside the cloud layer. The used scavenging ratios are: Λbc = 100000 and Λc = 700000.The total amount of pollutant scavenged by the wet deposition is then dependent on the actual height of the formation of the precipitation, and on the vertical concentration profile.SimpleBoxcited from [Brandes et al ., 1996]Value for the deposition mass transfer coefficient WASHOUT may be obtained by means of: ][][][S S S .SCAVratio RAINRATE = WASHOUTwith WASHOUT [S ] - mass transfer coefficient for wet atmospheric deposition at scale S [m air ·s -1] (D);RAINRATE [S ] - rate of wet precipitation at scale S [m rain .s -1] (A);SCAVratio [S ]- scavenging ratio (quotient of the total concentration in rainwater and the total concentration in air) of the chemical at scale S [-] (A).The scavenging ratio may be known from measurements or estimated:][][][][][1S S aerosol S water air S aerosol S COLLECTeff FRass K FRass SCAVratio +-=-with SCAVratio [S ] - scavenging ratio (quotient of the total concentration in rainwater and the total concentrationin air) of the chemical at scale S [-] (A); FRass aerosol [S ] - fraction of the chemical in air that is associated with aerosol particles at scale S [-] (A); K air-water [S ] - air-water equilibrium distribution constant at scale S [mol·m air -3/mol·m water -3] (A);COLLECTeff [S ] - aerosol collection efficiency at scale S [-] (A),The first term represents an estimate of the (equilibrium) distribution between rain water in air and the gas phase of air. The second term represents the scavenging of aerosol particles by rain droplets. The proportionalityconstant of 2⋅105is taken from Mackay [1991].Deposition mass flows of the chemical depend on the rate of wet precipitation. Collection efficiencies of aerosols vary greatly with the size of the particles. As chemicals may be associated with particles of a specific size, the collection efficiencies depends also on the chemical. The values given are typical values, to be used as a starting point:5][10.2 = COLLECTeff SwithCOLLECTeff [S ] - aerosol collection efficiency at scale S [-] (A ).Table C4. RAINRATE of the scales* - from Wania and Mackay [1995]CAM/POPsThe precipitation scavenging of POPs particles by falling rain or snow is coupled with the wet removal of aerosols in the CAM model.The particulate wet deposition flux, F p , can be written as:p snow or rain p C h F )(ψ-=,where h is the falling distance, C p is the particulate phase POPs concentration, ψ is the scavenging rate for rain or snow [Gong et al. 1997].The gas phase POPs are assumed to be in quasi-steady equilibrium with the rain drop. The air-water equilibrium coefficient, K aw , is a dimensionless partition coefficient that can be derived from Henry’s L aw constant, H(Pa ·m 3/mol) [Seinfeld , 1986].K aw = H / (R·T)and, H = H 0 exp (a(1/T 0 - 1/T)),where T is the temperature (K) and R is the gas constant (8.314 Pa ·m 3/mol ·K or J/mol/K), H 0 is the value at the reference temperature T 0, and a is a parameter of temperature dependence.The net wet deposition flux, F w , is then written as:G AW w C K p F ⋅-=)/(where p is the precipitation rate, usually reported in mm/h and C G is the gas phase POPs concentration.MSCE-POPWet deposition of particulate phaseThe values of concentration in precipitation are given by the formula:pap s w C W C =,where paC - the particle bound phase concentration in the air surface layer, ng/m 3; swC - the suspended phase concentration in precipitation water, ng/m 3;W p =1.5 ⋅ 105- the dimensionless washout ratio for the particulate phase.Wet deposition of gaseous phasegag d w C W C =,where dw C - the dissolved phase concentration in precipitation water, ng/m 3; gaC - the gaseous phase concentration in air, ng/m 3;W g = 1/K H - the dimensionless washout ratio for the gaseous phase; K H - the dimensi onless Henry’s law constant.C.4. Gaseous exchange between atmosphere and soil EVN-BETR and UK-MODELAir-soil diffusionD air-soil = (Soil Area · Z air ) / [(Z air / (MTC as · Z air + MTC sw · Z water )) + 1 / MTC sabl ] whereAverage soil depth = 10 cm; Soil Area = 8.36 1012m 2;Z water = Z air / K AW = 543 mol/m 3Pa;MTC as - soil air-phase diffusion transport velocity = 0.04 m/h; MTC sw - soil water-phase diffusion transport velocity = 1 x 10-5m/h; MTC sabl - soil air boundary layer transport velocity = 1 m/h.Air-soil rain dissolutionD air-soil = Soil Area · U R · Z waterCliMoChemcited from [Scheringer et al ., 2003]Diffusion from atmosphere to baresoil and vegetation-covered soil()gas gas i K foc rhoom regc K h gasS gas C V A vS K vL vG v Phi dtdC ow h⋅⋅⎪⎪⎭⎪⎪⎬⎫⎪⎪⎩⎪⎪⎨⎧+++--=-⋅⋅⋅1111Analogous to the procedure in subsection C.3, the parameter v gasS is changed if the year consists of exactly four seasons with varying temperatures (compare tables below).baresoil:vegetation-covered soil:The vegetation cover consists of three types: Grass, Coniferous Forest and Deciduous Forest. The variables VegGrass, VegCon and VegDec describe the fraction of the vegetation-covered soil occupied by the different cover types. Their numeric value is between 0-1 and depend on the climatic zone. Calculation of vG, vL, vS [Jury et al., 1983].When calculating the v i -value, the corresponding Di -value must be used (eg. for calculating vG, the DG-value is used):()⎥⎦⎤⎢⎣⎡⋅++--⋅⋅⋅⋅=h airsoil wsoil airsoil wsoil ow OC h h ii K frac frac frac frac K f rhoom regc K D v soil 12property sheets, See Chapter 3 and Annex B. ()⎪⎪⎭⎫ ⎝⎛+⋅=231043.0wsoil airsoil airsoil frac fracfrac DG()⎪⎪⎭⎫ ⎝⎛+⋅=2310000043.0wsoil airsoil wsoil frac fracfrac DL Calculation of the fraction of organic matter (f OC ) in the vegetation covered soilThe model contains three types of vegetation. For each type, the f OC is different (see table below). Depending onthe composition of a climatic zone, f OC is calculated as follows:co n d e c g r a ss OC i OC i OC i i OC f n fractionco f c fractionde f ass fractiongr f ⋅+⋅+⋅=fraction of organic matter in vegetation-covered soil in climatic zone i Diffusion from baresoil and vegetation-covered soil to the atmospheresoil gas i K f rhoom regc K h gasS K f rhoomregc frac K f rhoom regc K airsoil wsoil airsoil rhopart tsp partair rhopart tsp ow OC hgas C V A vSK vL vG v frac frac frac rhopart k K dC owOC how OC wsoil owOC h⋅⋅⎪⎪⎭⎪⎪⎬⎫⎪⎪⎩⎪⎪⎨⎧+++⎪⎪⎪⎭⎫ ⎝⎛⋅⋅+--⋅⋅+-⋅=-⋅⋅⋅⋅⋅⋅⋅⋅⋅11111For explanation of the grey part in the formula see subsection 1.。
《环境化学》教学中的多介质环境理念

Co nc e pt o f Mu l t i me d i a Env i r o nm e nt i n Env i r o n me n t a l Che mi s t r y Te a c hi ng
C I t I, l i e
( S c h o o l o f E n v i r o n m e n t a l S c i e n c e a n d T e c h n o l o g y , T i a n j i n U n i v e r s i t y , T i a n j i n 3 0 0 0 7 2 ,C h i n a )
Ke y wo r ds:En v i r o n me n t a l Ch e mi s t r y;t e a c h i n g c o n t e n t s;c o n c e pt o f mu l t i me d i a e n v i r o nme n t
《 环境化学》 是环境专 业学 生 的专 业必 修课 之一 。它是研 究化学 污染物质 在 环境 中的来 源 、分 布 、迁 移 、转 化 、效 应 、
第4 1 卷第 2 4期 2 0 1 3年 1 2月
广
州
化
工
Vo 1 . 4 1 No . 2 4 D e c e mb e r . 2 0 1 3
G u a n g z h o u C h e mi c a l I n d u s t r y
《 环境化学》 教学 中的多介质环境理念
s e l e c t e d t o e x p l a i n t h e t r a n s f e r b e h a v i o r s o f c h e mi c l a c o n t a mi n a n t s i n mu l t i me d i a e n v i r o n me n t .wh i c h wa s h e l p f u l f o r t h e s t u d e n t s i mp r o v i n g t h e i r a wa r e n e s s o f e n v i r o n me n t a l i s s u e s a n d a b i l i t y o f p o l l u t i o n c o n t r o 1 .
环境化学_多介质环境模型

内容
第一节 基本概念 第二节 污染物在环境中的迁移转化过程 第三节 多介质环境逸度模型的类型及计算
第四节 代表性的多介质环境逸度模型
内容
第一节 基本概念
一、多介质环境系统 二、稳态和平衡
三、逸度
一、多介质环境系统
环境介质 (Environmental Media), 相 (Phase,Compartment)
n ciVi ZifiVi
2、开放系统,稳态方程
Iin Iout
3、非稳态方程
dc / ct Iin Iout
二、多介质环境逸度模型的分级
1、Ⅰ级多介质环境逸度模型 Ⅰ级模型对应的是稳态、平衡、无流动环境系统,封闭环境
2、Ⅱ级多介质环境逸度模型 Ⅱ级模型对应的是稳态、平衡、有流动环境系统,开放系统 3、Ⅲ级多介质环境逸度模型 Ⅲ级模型对应的是稳态、非平衡、有流动环境系统 4、Ⅳ级多介质环境逸度模型 Ⅳ级模型对应的是非稳态、非平衡、有流动环境系统
一、污染物在环境中的迁移过程
2、扩散 分子热运动引起的热力学自发的增墒过程。 (1)相内的分子扩散
JA B
1
d A M dy
c
JA:A在单位面积上的扩散通量,mol· m-2· hBM:A在介质B中的分子扩散系数,m2· h-1 cA:介质B中A的浓度,mol· m-3 y: A分子在扩散方向上经过的距离,m dc A :A沿扩散方向的浓度梯度 dy
二、表层土壤模型
表层土壤模型可以用来模拟污染物在土壤中的降解、挥发和渗 滤等过程,评价各种迁移转化过程的相对重要性,污染物在 土壤中的持久性和最终归趋,以及预测各种修复措施使土壤 恢复到可接受的污染程度和所需要的时间。
多介质模型在海湾突发性有机污染中的应用及案例分析

突发性 海洋 污染 具有 发生 突然 、 污染 物扩 散快
1 环 境 多 介 质 模 型
和环境 危 害大等 特点 ¨ , 经 济 生 活 、 体健 康 和 对 人
生 态 系统 均 有 负 面 影 响 。 近 年 来 , 着 我 国社 随 会 生产 力和 科学 技术 的迅 速发 展 , 突发性 海洋 污染 事 故发 生 频 率 增 加 , 目前 海 洋 环 境 监 测 技 术 不 完 善 , 染物 的扩 散规 律与 危害 很难 在极 短 的时间 内 污
摘 要 : 弥 补 海 洋 环 境 监 测 能 力 不 足 , 用 受 潮 汐 动 力 控 制 海 湾 中 的 多 介 质 模 型 , 测 突 发 性 污 染 发 生 后 海 洋 环 境 为 利 预
中污 染 物 的浓 度 变 化 信 息 , 对 突 发 性 污 染 物 的迁 移 转 化 进 行 了计 算 。模 型 中使 用 逸 度 方 法 和 质 量 平 衡 算 法 , 测 非 挥 发 并 预
mu tme i d lf rb y o to ld b i a y a c su e o p e itpol t n o c n r to n s a wae , li d a mo e o a s c n r l y td ld n mi swa s d t r d c lu a tc n e tai n i e t r e
MO u c a J n- h o,ZHANG is e Ha —h ng,XUE Bi n,LI a — a,ZHANG in U Xio y Ha — a
( eodIstt o ca o rp y S A, aoaoyo r eE oyt n i ece s y Scn ntue fO en ga h , O L brtr Mai cs e a dBo ohmir, i f n sm g t S A, a ghu Z ea g3 0 1 ,C ia O H n zo , h in 10 2 hn ) j
环境化学第七章 多介质环境模型

三、多介质环境逸度模型的计算 3、模型构建和计算 、 4、模型验证 、 5、模型的灵敏度和不确定性分析 、 灵敏度: 灵敏度:模型输出对模型参数改变的响应 不确定性分析:模型输入的不确定性对预测结果可靠性的影响 不确定性分析:
第四节 代表性的多介质环境逸度模型
一、空气-水交换模型 空气 水交换模型 二、表层土壤模型 三、底泥-水交换模型 底泥 水交换模型 四、QWASI模型 模型 五、全球分布模型
一、污染物在4)在多孔介质中的扩散 ) (5)相间扩散过程 )
二、污染物在环境中的降解反应 对于整个环境而言, 对于整个环境而言,污染物只有通过降解反应才能真正的被 去除。描述污染物降解反应过程的动力学中, 去除。描述污染物降解反应过程的动力学中,应用最多的是 一级反应动力学: 一级反应动力学:
四、QWASI模型 模型 QWASI模型描述了点源排放、河水流入和大气沉降所引入的污 模型描述了点源排放、 模型描述了点源排放 染物在湖泊和河流中的多介质环境行为。 染物在湖泊和河流中的多介质环境行为。 1、湖泊中污染物归趋的QWASI模型 、湖泊中污染物归趋的 模型 该模型适用于水流和颗粒物流均匀混合的水体。 该模型适用于水流和颗粒物流均匀混合的水体。 2、河流中污染物归趋的QWASI模型 2、河流中污染物归趋的QWASI模型 3、多段 、多段QWASI模型 模型
一、污染物在环境中的迁移过程
环境中,污染物可以通过以下几种平流过程输入和输出: 环境中,污染物可以通过以下几种平流过程输入和输出: 1. 空气的输入和输出 2. 水的输入和输出 3. 水中存在的颗粒物和生物的输入和输出 4. 水从表层土壤渗入地下(地下水补给) 水从表层土壤渗入地下(地下水补给) 5. 空气中存在的气溶胶的输入和输出 6. 空气从对流层向平流层迁移,即空气的垂直运动 空气从对流层向平流层迁移, 7. 底泥的掩埋
《环境化学》(第二版)(戴树桂)知识点总结(共18页)-(1)

环境化学第一章绪论1、环境:环境是指与某一中心事物有关(相适应)的周围客观事物的总和,中心事物是指被研究的对象。
对人类社会而言,环境就是影响人类生存和发展的物质、能量、社会、自然因素的总和。
1972年,联合国在瑞典斯德哥尔摩召开了人类环境会议,通过了《人类环境宣言》.2、构成环境的四个自然圈层包括土壤、岩石圈、大气圈和水圈3、为保护人类生存环境,联合国将每年的4月22定位世界地球日,6月5日定位世界环境日。
4、环境保护的主要对象是由于人类生产、生活活动所引起的次生环境问题,主要包括:环境污染和生态破环两个方面。
5、环境问题:全球环境或区域环境中出现不利于人类生存和发展的各种现象,称为环境问题。
原生环境问题:自然力引发,也称第一类环境问题,火山喷发、地震、洪灾等。
次生环境问题:人类生产、生活引起生态破坏和环境污染,反过来危及人类生存和发展的现象,也称第二类环境问题。
目前的环境问题一般都是次生环境问题。
生态破坏:人类活动直接作用于自然生态系统,造成生态系统的生产能力显著减少和结构显著该变,如草原退化、物种灭绝、水土流失等。
当今世界上最引人注目的几个环境问题温室效应、臭氧空洞、酸雨等是由大气污染所引起的。
6、环境污染:由于人为因素使环境的构成状态发生变化,环境素质下降,从而扰乱和破坏了生态系统和人们的正常生活和生产条件。
造成环境污染的因素有物理、化学和生物的三个方面,其中化学物质引起的约占80%~90%.环境污染物定义:进入环境后使环境的正常组成和性质发生直接或间接有害于人类的变化的物质称为环境污染物。
污染物的性质和环境化学行为取决于它们的化学结构和在环境中的存在状态。
(五十年代日本出现的痛痛病是由镉Cd 污染水体后引起的;五十年代日本出现的水俣病是由 Hg 污染水体后引起的)重要污染物(1)元素:Cr,Hg,As,Pb,Cl(2)无机物:CO,NOx,SO2,KCN(3)有机化合物和烃类:烷烃(饱和)、芳香烃(苯环)、不饱和非芳香烃(不饱和,不带苯环)、多环芳烃(4)金属有机和准金属有机化合物: 四乙基铅、三丁基锡(5)含氧有机化合物:环氧乙烷、醚、醇、醛、酮、酚、有机酸等(6)有机氮化合物:胺、腈、硝基苯、三硝基苯(TNT)(7)有机卤化物:氯仿(四氯化碳)、PCBs、氯代二恶英、氯代苯酚(8)有机硫化合物:硫醇类(甲硫醇)、硫酸二甲酯(9)有机磷化合物:有机磷农药、磷酸二甲酯、磷酸三乙酯按受污染物影响的环境要素可分为大气污染物、水体污染物、土壤污染物等;按污染物的形态可分为气体污染物、液体污染物和固体废物;按污染物的性质可分为化学污染物、物理污染物和生物污染物.优先控制污染物:概念:基于有毒化学物的毒性、自然降解的可能性及在水体中出现的概率等因素,从多种有机物中筛选出的优先控制物7、认识过程: 20世纪60年代人们只把环境问题作为污染来看待,没有认识到生态破坏的问题。
环境介质

苯并(a)芘的环境多介质迁移和归趋 苯并 芘的环境多介质迁移和归趋 模拟
将某地区的环境相分为4个主相,即大气、 水体、土壤和沉积物,下标依次为1、2、3、 4;每个主相中又部分包括气、固和水等子 相。大气中包括气相和气溶胶;水体中包 括水、悬浮物、鱼(代表水生生物);土壤中 包括气相、固相和水相;沉积物中包括固 相和水相。根据稳态假设和质量平衡关系 可以建立涉及主相迁移量的平衡表达式,
需要收集的数据收集的数据包括模型验证的数据和用于模型输入的数据模型的计算过程共涉及到63个输入参数分为13个描述苯并a芘物理2化学性质的参数44个区域环境参数和6个表征系统输入量的参数大气和水平流过程中的输入浓度则取本地区的实测值苯并a芘在研究区域的来源当地系统向环境相中的排放和系统外大气和水的长距离平流输送工业燃油交通燃油工业含发电供热燃煤炼焦用煤家庭燃煤及天然气等
多介质归趋模型
我们把某种物质在各种介质中的持续 和运移统称为该物质在介质中的环境 归趋, 这种归趋不仅取决于农药本身固 有的性质(如蒸气压、水溶度、生物活 性等) , 而且还与外部的环境因素(如介 质性质和气候条件等) 密切相关,综合 考虑这些得出的一种模型即称为多介 归趋模型。
பைடு நூலகம்
归趋模型的背景
进入环境中的有机物,对动植物的危害性。 如果其进入环境后,可被分解为毒性小或 无毒,则对这类有机物处理得当,那么, 它就不属于禁用或严格控制之列,不但不 属于污染物,而且还是工农业生产的资源。 因此,研究水、土环境中各种有机毒物的 预测模型是一个十分重要的问题。预测归 趋模型可以帮助我们预测污染物在环境中 浓度的分布及通过各种迁移转化过程后的 最终归趋。
主讲人: 主讲人:eric 2011.1.4
一、环境介质的定义 二、多介归趋模型的介绍 苯并(a)芘归趋模型 三、苯并 芘归趋模型 四、环境多介质模型的发展
农药在环境中的多介质模型及其研究进展

安徽农 业科 学 . u ao A hi giSi20 , 4 2 )5 1,3 3 J r l f nu A r c 06 3 (0 :3 15 1 o n . .
责任 编辑 姜 丽 责任 校对 胡剑胜
农 药 在环 境 中的 多介质 模 型 及 其研 究进 展
难从 整体上 认识 环境 介质 变 化规 律及 农药 分 布状 态 ,同时
1 迁移 。 2
包括混合并稀释 、 平流和对流 、 扩散和弥散等。
自然界中有 2 种迁移现象: ①随机运动迁移 ; ②定向运动迁 移 。 种 迁移 都可 以从分 子水 平到 全球距 离 , 微秒 到地质 2 从
年代 在较 宽 的尺度 范围 上发 生 。上 面提 到的扩 散 和弥散 都
究 进展 。
的了解 , 要做到对真实环境体 系的有效预测是完全可能的。
运 用多 介质循 环 模型对 环 境 中农药 污 染进 行 预测 ,可 以求 得 农药 在环 境 系统各介 质 中 的浓度 分 布 、 种 迁移 转化 作 各 用 的过 程速 率 以及积 累量 、滞 留时 间等 重要 的 环境 化学 参 数。 同时 , 多介 质循 环模 型 还可 以对一 种新 合成 农药 的 运用 环境 效应 进行 评价 , 而 达到 防治 污染 的 目的 , 从 为农 药的 管 理 和评价 提供 了一 个有 力 的技术 方法 和手段 。 3 多 介质环 境模 型的研 究 现状及 发展
的残留量 , 至在 常年 不化 的冰层 中也检 出 0 4 g g 甚 . / 滴滴 0nk
涕 。因此 , 究农药 在 多介质 环 境 中的迁 移 、 化 和预 测是 研 转 十 分必要 的… 。
2 多介质模 型 的定 义与 建模 的意 义 农 药在 多介质 环境 中的循环 模 型是 研究 多 介质 环境 中 介 质 内及介质 单元 间农 药迁 移 、转 化和 环境 归趋 定 量关 系 的数 学 表达式 ,其 主要 特点 是 可 以将各 种不 同 的环 境介质 同导 致污 染物 跨介 质单 元边 界 的各 种过 程相 连接 ,并能在 不 同模 型结构 的水 平上 对这 些过程 公式 化 、 量化 。 定 这 类模 型虽 然数 学形 式 较为 复杂 , 然而 , 只要 对介 质 内 及介质 问农 药 的物理 、化 学性 质 和生 物过 程进 行较 为深入
环境多介质模型综述

环境多介质模型综述摘要:环境多介质模型可用于综合描述化合物在实际环境中的分配和迁移过程,是进行污染物环境归趋模拟和环境风险评价的有效工具。
本文在介绍了多介质模型类型的基础上,概述了部分多介质模型的结构和建模方法,并对其应用前景和发展趋势做了展望。
关键词:多介质环境模型;环境归趋;前景与展望环境中存在的各类污染物,其环境残留的潜在影响成为了公众健康优先考虑的问题之一。
为了解决这些污染物所造成的环境问题,测定其在环境中的污染水平是很有必要的,但是若对这些污染物在各种环境介质中的时空分布情况进行一一监测会消耗大量的人力和物力,可行性较低。
所以人们尝试建立数学模型来解决此问题。
上世纪50年代人们在大气、水或土壤等单一环境介质中建立了一些简单模型和公式来预测污染物在这些介质中的分配和归趋。
这是早期的对污染物环境分配行为的建模研究,属于单介质模型。
图1环境中污染物质的迁移循环我们生存的环境是由水、气、土、植物、动物等组成的一个联系紧密复杂的多介质系统,一般来说,污染物特别是有机污染物排出之后,不会固定在某一位置,而是要发生稀释、扩散、降解等一系列物理、化学、生物等过程,在各环境介质中进行迁移、转化和分配。
人们需要一个新颖的方法,在跨区域的多元环境介质之间以及大陆和全球范围下,研究化学品迁移的广泛特性。
也就是说,对环境污染物的研究不仅要考虑到在一个介质中化学物质的迁移和转化,而且还要考虑污染物在环境介质之间的迁移速率以及在土壤/水/空气界面水文地质过程。
图1简要描述了在环境中普遍的污染物质的迁移循环,在此应用背景下多介质环境模型的研究一经提出,就得到了迅速的发展和广泛的应用。
本文在介绍了多介质模型类型的基础上,概述了部分多介质模型的结构和建模方法,并对其应用前景和发展趋势做了展望。
1 常见多介质模型类型及构建方法多介质模型建模的基本结构如下图2所示,目前的环境多介质模型主要有如下几种:基于逸度方法的环境多介质模型、综合多介质空间分区模型(ISMCM)、单一介质空间链接模型(LSSMM)模型、基于轨迹计算的概率模型、基于神经网络与灰色理论的参数预测模型[7]等等。
环境化学复习总结全部(戴树桂)

第三章:水环境化学第一节:天然水的基本特征及污染物的存在形式1.水中八大离子:K+,Ca+,Na+,Mg+,HCO3-,NO3-,Cl-,SO4(2-)2.气体在水中的溶解度服从Henry定律:一种气体在液体中的溶解度正比于液体所接触的该种气体的分压。
溶解度【X(aq)】=K H×p G K H为气体一定温度下Henry定律常数,p G分压3.氧在水中的溶解度CO2的溶解度P150页4.:BOD(生化需氧量):在一定体积水中有机物降解所需消耗的氧的量。
BOD5=DO1-DO55.碳酸平衡P152-P157计算题重点区域★★★6.水中污染物的分布和存在形态:A.有机污染物:农药(有机氯、磷,氨基甲酸醇),多氯联苯PCBs,卤代脂肪烃,醚类,单环芳香族化合物,苯酚类和甲酚类,钛酸酯类,多环芳烃PAH,亚硝胺和其他化合物B.金属污染物:镉,汞,铅,砷,铬,铜,锌,铊等7.优先污染物:有毒物质品种繁多,在众多的污染物中筛选出潜在危险大的作为优先研究和控制对象。
8.水中的营养元素:N,P,C,O和微量元素9.水体富营养化:生物所需的N,P等营养物质大量进入湖泊,河口等缓流水体,引起藻类及其他浮游生物迅速繁殖,水体溶解氧量下降,鱼类及其他生物大量死亡的现象。
10.N/P>100,贫营养化;N/P<10,富营养化;第二节:水中无机污染物的迁移转化一,颗粒物与水之间的迁移:1水中颗粒物类别:矿物微粒和黏土矿物,金属水合氧化物,腐殖质,水体悬浮沉积物2.水环境中胶体颗粒物的吸附作用类别:表面吸附,离子交换吸附,专属吸附。
3.表面吸附:胶体具有巨大的比表面积和表面能,因此固液界面存在表面吸附作用,属于物理吸附。
4.离子交换吸附:环境中大部分胶体带负电荷,容易吸附阳离子,在吸附过程中,胶体每吸附一部分阳离子,同时也放出等量的其他阳离子。
5.专属吸附:除了化学键的作用外,尚有加强的憎水键和范德华力或氢键在起作用。
《环境化学》考试大纲

《环境化学》考试大纲一、考试大纲的性质环境化学是环境科学与工程专业的专业基础课,也是报考环境科学与工程学科的考试科目之一。
为帮助考生明确考试复习范围和有关要求,特制定出本考试大纲。
本考试大纲主要依据北京林业大学本科环境化学教学大纲编制而成,适用于报考北京林业大学硕士学位研究生的考生。
二、考试内容第一章水环境化学(1) 天然水的基本特征,水中污染物的分布和存在形态,水中营养元素及水体富营养化(2) 颗粒物与水之间的迁移,水中颗粒物的聚集,溶解和沉淀,氧化还原,配合作用(3) 分配作用,水解作用,光解作用,生物降解作用,挥发作用(4) 氧平衡模型,湖泊富营养化预测模型,有毒有机污染物的归趋模型,多介质环境数学模型基本要求:该部分主要内容涉及介绍天然水的基本特征,水中重要污染物存在形态及分布,污染物在水环境中的迁移转化的基本原理及水质模型。
要求了解天然水的基本性质,掌握无机污染物在水环境中进行沉淀-溶解、氧化还原、配合作用、吸附-解吸、絮凝-沉降等迁移转化过程的基本原理,并运用所学原理计算水体中金属存在形态,确定各类化合物溶解度,以及天然水中各类污染物的pE计算及pE-pH图的制作。
了解颗粒物在水环境中聚集和吸附-解吸的基本原理,掌握有机污染物在水体中的迁移转化过程和分配系数、挥发速率、水解速率、光解速率和生物降解速率的计算方法,了解各类水质模型的基本原理和应用范围。
第二章大气环境化学(1) 大气的主要成分、大气层的结构、大气中的主要污染物〔重点、难点内容〕(2)影响大气污染物迁移的因素(3) 自由基化学基础、光化学反应基础、大气中重要的自由基来源、氮氧化物的转化、碳氢化合物的转化、光化学烟雾、硫氧化物的转化及硫酸烟雾型污染、酸性降水、温室气体和温室效应、臭氧层的形成与耗损(4) 大气颗粒物的来源与消除、大气颗粒物的粒径分布、大气颗粒物的化学组成、大气颗粒物的来源识别、大气颗粒物中的PM基本要求:该部分主要内容涉及大气结构,大气中的主要污染物及其迁移,光化学反应基础,重要的大气污染化学问题及其形成机制。
《环境化学课件》PPT课件

水体污染
工业废水、生活污水等 排放到水体中的污染物 ,通过饮水和食物链进
入生物体。
土壤污染
农药、重金属等污染物 在土壤中积累,通过食
物链进入生物体。
其他途径
包括食物摄入、皮肤接 触等途径,使污染物进
入生物体。
生物体内污染物质转化与排泄机制
01
生物转化
生物体内的酶系统可将污染物转化为低毒或无毒的代谢产物,如肝脏中
化学法
包括中和、氧化还原、电解和混凝等。
生物法
利用微生物的代谢作用,将有机物转化为无害物质,包括活性污泥 法、生物膜法和厌氧生物处理法等。
土壤污染治理技术与方法
物理化学修复技术
包括土壤淋洗、土壤固化/稳定化、热脱附和电动 修复等。
生物修复技术
利用微生物或植物对土壤中的污染物进行降解、 转化或固定,包括微生物修复和植物修复等。
REPORT
《环境化学课件》 PPT课件
CATALOG
DATE
ANALYSIS
SUMMARY
目录
CONTENTS
• 环境化学概述 • 大气环境化学 • 水环境化学 • 土壤环境化学 • 生物体内污染物质运动过程及毒性效
应 • 环境污染治理技术与方法
REPORT
CATALOG
DATE
ANALYSIS
危害
影响水生生物生存、破坏水生态平衡、危害人类健康等。
水环境化学原理
1 2
水中的化学反应
酸碱反应、沉淀溶解反应、氧化还原反应等。
水质指标与标准
pH值、溶解氧、化学需氧量、生物需氧量等。
3
水处理原理与技术
物理处理、化学处理、生物处理等。
REPORT
多介质--利用多介质模型研究有机氯农药的环境行为

有具有随机特征的输入参数在输入时作了同步随机化
处理 ,模型计算结果 (10 000次) 给出了模型不确定性
信息 。所有的输入参数均为标准正态分布 ,模型求得
2 研究方法
211 模型的框架 研究区域划分为大气 、水和沉积物三个主相 ,每个
主相又包括气 、水 、固等子相 。考虑到鱼体中有机氯农 药的吸收来自水体 ,所以模型中将鱼视为水的生物子 相 。同时把整个汇水盆地地表水的输入作为整个小海 系统污染物的源 。考虑到小海海域面积的复杂性 ,本 文把小海作为一个系统来研究 ,假设在系统内各种污 染物的浓度混合均匀 。为了消除空间的差异性 ,用所 采集的 9 个点的样品的平均浓度来代表小海的浓度水 平 。采样点分布见图 1 。
目前 ,学者们解决问题时大部分只局限在单一的 环境介质单元内 ,很难从整体上认识环境介质变化规 律及农药分布状态 ,同时也难以提出控制污染的有效 途径 。为解决持久性有机污染物的各种环境和生态问 题 ,建立快速 、准确的实时监测方法 ,测定持久性有机 污染物在环境各介质中的污染水平 ,是非常重要的 。 因此 ,研究者越来越关注污染物在多种介质的环境行 为 ,特别是跨界面的迁移特征 ,多介质环境模型在系统 模拟有机物迁移方面的优势逐渐显示出来[5~8] 。本文 主要通过研究小海湾地区有机氯农药在海水 、沉积物
312 有机氯农药在各相中的分布 模型的计算结果表明 ,在小海大气 、海水和沉积物
中 , p , p’2DDT 的浓度分别为 :01019 8gΠm3 、01016 8ngΠ L 、01313ngΠg ; p , p’2DDE : 01019 9 gΠm3 , 、01001 65 ngΠL 、 01124ngΠg ; p , p ’2DDD : 01001 98 gΠm3 、01004 68 ngΠL 、 01083 2ngΠg。从图 3 可以看出 ,三种有机氯农药在沉 积物中的浓度最高 ,占总环境滞留量的 99 %。这主要 是由于沉积物有机质含量高 ,且没有平流输出作用 ,对 有机氯农药具有很强的吸附作用 。3 种有机氯农药在 大气相中 ,颗粒物的浓度比大气高很多 ,但基于其微小 的比例 ,其滞留量仅占总量的 01211 % (以 p , p’2DDT 为例) 。而大气中的富集比例比大气中的悬浮物高出 1 个数量级以上 ,即 4117 % ; 水中悬浮物和生物的浓 度比水的高 ,特别是悬浮物的浓度比水的浓度高出 4 个数量级 ,但以水占绝对的优势 ,滞留量比悬浮物高出 1 个数量级以上 。沉积物颗粒物吸附有机氯农药比空 隙水多得多 ,滞留量占总量的 99 %以上 ,是小海中有 机氯农药最主要的“汇”。如果在评估区域有机氯农药 的总量的时候 ,可以直接以沉积物相代替 ,其他相可以 忽略 。虽然大气和水吸附的量在总滞留量中比例很 低 ,但考虑到可能直接通过食物或者呼吸进入人体 ,仍 能对人体健康构成威胁 。
环境多介质归趋模型简介

一般而言,真实的环境系统和我们模拟的环
境系统之间存在一定的差距,在构建模型时,
需要用一系列的假设来简化真实的环境系统,
比如:
环境体系由多个环境主相和若干子相组成;
任一时刻,每个环境主相的污染物呈均匀分
布,各子相之间的逸度关系符合平衡稳态,即
逸度值在同一时刻处处相等;
•2/13/2024 环境主相之间处于不平衡动态。
•2/13/2024
•34
Ⅳ级模型
Ⅳ级模型系统是非平衡、非稳态、流动系 统。它假定物质在各相间处于非平衡状态, 考虑物质的非稳态输入与输出和在相内发生 的各种反应,以及物质在各相间的扩散与非 扩散过程。该系统能很好地描述污染物在环 境系统中的动态行为。
•2/13/2024
•35
多介质环境模型计算的基础
•2/13/2024
•12
多介质模型的建立
•2/13/2024
•13
(一)模型概化 (二)污染物的环境迁移过程 (三)模型参数 (四)模型构建和计算 (五)模型验证
•2/13/2024
•14
(一)模型概化
首先应该确定研究区域,包括研究区域的地
理位置和范围。然后要明确研究区域所涉及的
多介质环境,划分基本的环境相和子相。
•2/13/2024
•27
•2/13/2024
•28
(五)模型的验证
由于环境的复杂性和可变性,多介质环 境模型只能是对真实环境的简化,模型输 出只能是对污染物归宿的近似模拟,因此 模型结果的验证过程非常重要。用实测数 据对模型结果进行验证,如果结果差值在 一个数量级之内,表明模型结果能够比较 客观地描述污染物的多介质环境行为,如 果结果偏差较大,则需要对模型调整后重 新计算。
环境化学_1 环境介质及其性质

一、大气温度层结
对流层
[ O3 + hv O + O2 ] Troposphere n. [气] 对流层 高度为10~12米,温度变化特点是: 200-300 nm, 300-360 nm 通常0.6K/100m;气体垂直运动激 λmax = 254 nm 烈;含有大气全部质量的3/4和几 乎全部水气,复杂的天气现象 (15 O + O2 + M O3 + M,热量 ℃~-56 ℃)。 平流层 Stratosphere [ ] n. [气] 同温层 高度为10~50 km;温度随高度 的增高而递增;垂直对流少,大 气稳定,(-56 ℃ ~-2 ℃)。 中间层 Mesosphere [ ] 高度为48~78 km;气温 随高度的增加而降低;空 气运动激烈 (meso-)
85 km -92 ℃ O2+, NO+ Ultraviolet: λ 200-330 nm, penetration to ~ 50 km
High energy ultraviolet: λ<100 nm, Penetration to ~ 1200 km
1200 km, [O]=[O2]
Mesosphere
积物、湖中悬浮物及水或土壤中的生物体等。 连续的介质或相:水、大气...
非连续的介质或相:大气颗粒物…
相邻的环境介质或相:水体-大气(污染物迁移)、...
非相邻的环境介质或相:大气-水体沉积物…
二、环境介质(相)
容易受到污染的介质或相:表层水、大气...
不容易受到污染的介质或相:深海、岩石层… 均相:如浅塘中充分混合的水,无浓度梯度、温度梯度;
的颗粒物的总质量,通常称为总悬浮颗粒物。用TSP表示。其
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
1、湖泊中污染物归趋的QWASI模型 该模型适用于水流和颗粒物流均匀混合的水体。 2、河流中污染物归趋的QWASI模型 3、多段QWASI模型
五、全球分布模型
三、底泥-水交换模型
该模型可以用来估算污染物被底泥积累或释放的速率、底栖生 物体内污染物的浓度,评价污染物不同迁移过程的重要性以 及污染恢复时间。
该模型有以下几个过程: 底泥沉降;底泥再悬浮、底泥掩埋、水体和空隙水间的扩散交 换、底泥中的反应、灌溉。
四、QWASI模型
QWASI模型描述了点源排放、河水流入和大气沉降所引入的污 染物在湖泊和河流中的多介质环境行为。
三、多介质环境逸度模型的计算
1、模型的基本结构 构建模型时需要用假设来简化真实的环境系统: (1)环境体系由多个环境主相和若干子相组成 (2)任一时刻,每个环境主相的污染物呈均匀分布,各子相 之间的逸度关系符合平衡稳态,逸度值在同一时刻处处相等。 (3)环境主相之间处于动态不平衡状态
2、模型输入参数 (1)环境参数 (2)污染物的性质数据 (3)污染物排放信息
环境的组成非常复杂,由一系列彼此相连的环境介质或
环境相来组成,如大气、土壤、湖泊、河流、海洋、湖底沉
积物、湖中悬浮物及水或土壤中的生物体等。 多介质环境系统:具有两种或两种以上环境介质的系统。 均相:如浅塘中充分混合的水,无浓度梯度、温度梯度; 非均相:性质不均一 (如土壤、沉积物)
二、稳态和平衡
稳态(stable):所研究体系中各个相的性质和状态是恒定
的,不随时间变化。
平衡(equilibrium):系统中每一相的浓度(或温度、压力) 保持稳定,而且化合物没有在各相间发生质量净迁移的趋势。
注意稳态和平衡的区别。
三、逸度
逸度:是一个热力学量,指实际气体对理想气体的校正压力, 或者说是物质脱离某一相的倾向的大小,用 f 表示,可以作 为判别污染物在环境各相间是否达到平衡的一个标准。 对理想气体与稀溶液,有: c=Z× f c:污染物的浓度,mol· m-3 f: 逸度,Pa Z:逸度容量,mol· m-3· Pa-1
Z值的计算,见下表。
三、逸度
环境相 空气 水 辛醇 类脂 气溶胶 有机碳 有机质 Z值/( mol· m-3· Pa-1 ) ZA = 1/(RT) ZW = 1/KH = ZA/KAW ZO = ZWKOW Z L = ZO ZQ = KQAZA ZOC = KOCZW(ρoc/103) 各符号的物理意义 R:摩尔气体常数 T:绝对温度 KH:亨利定律常数KAW:气/水分配系数 KOW :正辛醇/水分配系数 KQA :气溶胶/空气分配系数 KOC:土壤吸附系数,L· Kg-1 ρoc:有机碳的密度,103 Kg· m-3
ct c0 exp(kt b)
t 12 0.693 / k
第三节 多介质环境逸度模型的类型及计算
一、多介质环境逸度模型的基本原理
二、多介质环境逸度模型的分级
三、多介质环境逸度模型的计算
一、多介质环境逸度模型的基本原理
质量守恒是建立多介质环境模型最基本的原理。 质量平衡方程分为三类: 1、封闭系统,稳态方程
第七章 多介质环境模型
内容
第一节 基本概念 第二节 污染物在环境中的迁移转化过程 第三节 多介质环境逸度模型的类型及计算
第四节 代表性的多介质环境逸度模型
内容
第一节 基本概念
一、多介质环境系统 二、稳态和平衡
三、逸度
一、多介质环境系统
环境介质 (Environmental Media), 相 (Phase,Compartment)
f I / GiZi
f 表示污染物达到平衡时的逸度,i 代表空气、水等环境相。 如果污染物的总物质的量nT,其每个分子在该稳态系统中的
停留时间为:τ= nT /I 停留时间(滞留时间):污染物在某特定环境系统中存在时 间的长短,反映了污染物在环境中的持久性。
一、污染物在环境中的迁移过程
环境中,污染物可以通过以下几种平流过程输入和输出: 1. 空气的输入和输出 2. 水的输入和输出 3. 水中存在的颗粒物和生物的输入和输出 4. 水从表层土壤渗入地下(地下水补给) 5. 空气中存在的气溶胶的输入和输出 6. 空气从对流层向平流层迁移,即空气的垂直运动 7. 底泥的掩埋
n ciVi ZifiVi
2、开放系统,稳态方程
Iin Iout
3、非稳态方程
dc / ct Iin Iout
二、多介质环境逸度模型的分级
1、Ⅰ级多介质环境逸度模型 Ⅰ级模型对应的是稳态、平衡、无流动环境系统,封闭环境
2、Ⅱ级多介质环境逸度模型 Ⅱ级模型对应的是稳态、平衡、有流动环境系统,开放系统 3、Ⅲ级多介质环境逸度模型 Ⅲ级模型对应的是稳态、非平衡、有流动环境系统 4、Ⅳ级多介质环境逸度模型 Ⅳ级模型对应的是非稳态、非平衡、有流动环境系统
一、污染物在环境中的迁移过程
2、扩散 分子热运动引起的热力学自发的增墒过程。 (1)相内的分子扩散
JA B
1
d A M dy
c
JA:A在单位面积上的扩散通量,mol· m-2· hBM:A在介质B中的分子扩散系数,m2· h-1 cA:介质B中A的浓度,mol· m-3 y: A分子在扩散方向上经过的距离,m dc A :A沿扩散方向的浓度梯度 dy
三、逸度
根据估算Z值的方法,遵循以 f 值作为判别平衡标准的原 则,就可以进行多介质平衡分配的计算。
例题见教材p298页。
第二节 污染物在环境中的迁移转化过程
一、污染物在环境中的迁移过程
二、污染物在环境中的降解反应
一、污染物在环境中的迁移过程
1、平流 环境中发生的平流过程是由于污染物存在于一种流动的介质 中而随之进行的直接迁移过程。 污染物在环境介质中发生平流过程的计算: p299-300
一、污染物在环境中的迁移过程
(2)相内湍流或涡流扩散 (3)非稳态扩散 (4)在多孔介质中的扩散
(5)相间扩散过程
二、污染物在环境中的降解反应
对于整个环境而言,污染物只有通过降解反应才能真正的被 去除。描述污染物降解反应过程的动力学中,应用最多的是 一级反应动力学:
dc / dt kc
二、表层土壤模型
表层土壤模型可以用来模拟污染物在土壤中的降解、挥发和渗 滤等过程,评价各种迁移各种修复措施使土壤 恢复到可接受的污染程度和所需要的时间。
土壤中有四个相:空气、水、有机质、矿物质。
1、降解过程 2、渗滤过程 3、挥发沉降
三、多介质环境逸度模型的计算
3、模型构建和计算
4、模型验证 5、模型的灵敏度和不确定性分析 灵敏度:模型输出对模型参数改变的响应 不确定性分析:模型输入的不确定性对预测结果可靠性的影响
第四节 代表性的多介质环境逸度模型
一、空气-水交换模型
二、表层土壤模型
三、底泥-水交换模型
四、QWASI模型
五、全球分布模型
一、空气-水交换模型
空气-水交换模型可以用来估算污染物在污水处理池、池塘、湖 泊中的损失,污染物在大气中的沉降速度,从而解释空气和 水中污染物浓度变化趋势以及确定其迁移方向和速度。
该模型中考虑四个传质过程: 1、空气-水界面的扩散过程、蒸发作用和吸收过程 2、污染物随气溶胶的干沉降过程 3、污染物随降雨的湿沉降 4、污染物随气溶胶的湿沉降
ZOM = KOMZW(ρoM/103) KOM:有机质分配系数,L· Kg-1 ρoM:有机质的密 度,103 Kg· m-3 ZMM = KMMZW(ρMM/103) ρMM:矿物质的密度,103 Kg· m-3 KMM:矿物质分 配系数,L· Kg-1
矿物质
生物相
ZB = LZL
L:类脂的体积分数
