CE - The Time Value of Money
Chapter 3 The Time Value of Money 货币的时间价值 财务管理(双语版) 教学课件

年金现值:PVAn(Present Value of an Ordinary Annuity)
n 1
11i n
PV nR A t 11it R i
R PVi,nI
PVIFAi,n 年金现值系数(the Present Value Interest Factor of an Annuity at i for n periods)
P0V P 0FnV 1inFnV PVi,nIF
7. PVIFi,n : (the Present Value Interest Factor at i for n
periods)
8.
复利现值系数,利率为i,期数为n
6. Annuities 年金
系列、等额的收付
Ordinary Annuity 普通年金 收付款发生于每期期末 Annuity Due 预(即)付年金 收付款发生于每期期初
Perpetuity 永续年金
无限期支付的普通年金
Ordinary Annuity(普通年金)
0
R
R
R
R
年金终值:FVAn(Future Value of an Annuity)
FV nR A t n11intR1iin1RFVi,nIFA
FVIFAi,n 年金终值系数(the Future Value Interest Factor of an Annuity at i for n periods)
1
每年计息一次时产生的利息=名义利率每年计息m次时产生的利息
FV n A R F DV i,n 1 I 1 FA
现值 (Present Value)
比普通年金少折现一期
PV n A R P D V i,nI 1 F i A
货币时间价值概述

货币时间价值概述货币时间价值(Time Value of Money,简称TVM)是金融学中一个重要的概念,指的是货币在不同时间点的价值不同。
简单来说,TVM认为一笔现金在现在的价值大于同样一笔现金在未来的价值,因为它可以用于投资或者收益。
TVM的核心原理是时间的价值,即货币的价值随着时间的推移而增加或减少。
这是因为货币可以通过投资而产生利息、股息或其他盈利方式,也可以通过通货膨胀而贬值。
因此,对于投资者和借款人来说,了解和应用TVM原理是做出明智的金融决策的基础。
TVM的基本思想是将货币的价值量化为现值和未来值。
现值指的是一个金额在当前时间点的价值,未来值指的是相同金额在未来某一时间点的价值。
TVM涉及到现金流量的时间推移和调整,包括现金的未来价值、现金流量的折现、年金等。
具体来说,TVM包括以下几个重要概念和公式:1. 未来值(Future Value,简称FV):指的是将一笔现金在未来某一时间点的价值,可以通过对当前现金的投资来获得。
计算未来值的公式为:FV = PV * (1 + r)^n,其中PV代表现值,r代表年利率,n代表时间期限。
2. 现值(Present Value,简称PV):指的是一笔未来现金在当前时间点的价值,可以通过将未来现金流折算为当前现金来计算。
计算现值的公式为:PV = FV / (1 + r)^n。
3. 年金(Annuity):指的是在一段连续的时间内,以相同金额、相同时间间隔进行的现金流量。
年金可以是普通年金(Ordinary Annuity)或者永续年金(Perpetuity)。
普通年金的现值公式为:PV = P * [1 - (1 + r)^(-n)] / r,其中P代表每期支付的金额,r代表年利率,n代表支付期数。
4. 折现率(Discount Rate):指的是将未来现金流折算为现值时所使用的利率。
折现率通常是基于风险和机会成本等因素确定的。
TVM的应用广泛,包括投资决策、贷款计算、退休规划等方面。
时间价值的英语作文

时间价值的英语作文英文回答:The concept of time value of money (TVM) refers to the idea that money has a different value at different pointsin time. This is because money can be invested and grow over time, or it can be borrowed and accrue interest, meaning that the value of money changes depending on the time frame considered.There are several key concepts related to TVM:1. Present Value (PV): The present value of a future sum of money is the amount of money that would need to be invested today at a given interest rate to grow to that future value.2. Future Value (FV): The future value of a present sum of money is the amount of money that it will grow to at a given interest rate over a specified period of time.3. Interest Rate (r): The interest rate is the percentage of the principal amount that is charged or earned over a specific period of time.4. Time (t): Time is the period over which the money is invested or borrowed.The relationship between these concepts can be expressed mathematically using the following formula:FV = PV (1 + r)^t.This formula allows us to calculate the future value of a present sum of money, or the present value of a future sum of money, given the interest rate and the time period.中文回答:时间价值。
资金的时间价值计算

资金的时间价值计算资金的时间价值(Time Value of Money)是指在一定时间期限内,一笔资金的价值随着时间的推移而发生变化的现象。
简单来说,由于时间的推移会导致资金的价值增加或减少,因此相同金额的资金在不同时间点具有不同的价值。
例如,100元今天的价值可能高于100元明天的价值,因为你可以立即利用这100元进行投资或消费。
在金融学领域,资金的时间价值是一个重要的概念,它和利率、时间期限、现金流量等因素密切相关。
正确计算资金的时间价值对于金融决策和投资决策至关重要。
下面将介绍如何计算资金的时间价值:一、未来价值(Future Value,FV)的计算未来价值是指一笔资金在未来一些时间点的价值。
通过复利公式可以计算未来价值:FV=PV×(1+r)^n其中,FV代表未来价值,PV代表现值(Present Value),r代表年利率,n代表时间期限。
举个例子,假设你有一笔1000元的资金,想知道在5年后的未来价值。
假设年利率为5%。
将数据代入公式可以得到:FV=1000×(1+0.05)^5=1282.28元所以,在5年后,这笔1000元的资金的未来价值将增加到1282.28元。
二、现值(Present Value,PV)的计算现值是指将未来其中一时间点的金额折算到现在的价值。
一般情况下,人们更乐意拥有现金而非未来的同等金额,因为现金可以立即使用或投资。
通过贴现公式可以计算现值:PV=FV/(1+r)^n其中,PV代表现值,FV代表未来价值,r代表年利率,n代表时间期限。
举个例子,假设你知道在5年后,你需要1,500元来支付项费用,想知道如何将将来的金额折算为现在的价值。
假设年利率为5%。
将数据代入公式可以得到:PV=1500/(1+0.05)^5=1107.03元所以,如果你希望在5年后拥有1500元,现在就需要有1107.03元。
三、现金流量的折现计算现金流量是指一系列不同时间点的现金流入或流出。
财务管理基础课件:The Time Value of Money

Where FV = Future value PV = Present value i = Interest rate n = Number of periods;
In the previous case, PV = $1,000, i = 10%, n = 4, hence;
1st year……$1,000 X 1.10 = $1,100 2nd year…...$1,100 X 1.10 = $1,210 3rd year……$1,210 X 1.10 = $1,331 4th year……$1,331 X 1.10 = $1,464
9-4
Future Value – Single Amount (Cont’d)
• The time value of money is used to determine whether future benefits are sufficiently large to justify current outlays
• Mathematical tools of the time value of money are used in making capital allocation decisions
annuity
9-25
Yield – Present Value of a Single Amount
• To calculate the yield on an investment producing $1,464 after 4 years having a present value of $1,000:
Table 9–5
财务管理专业英语-The Time Value of Money
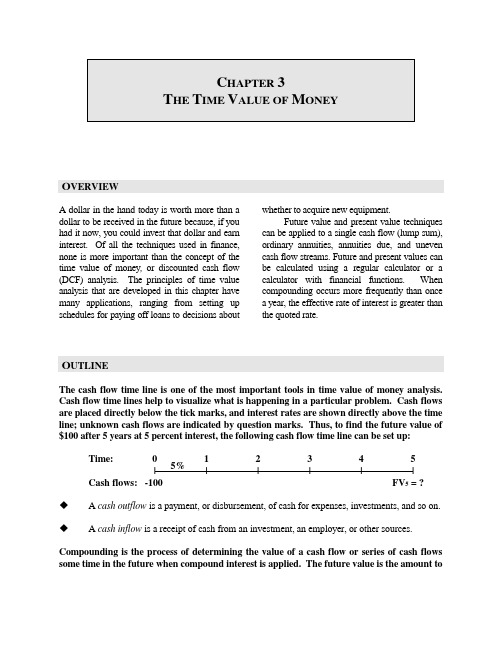
5 years: PV = 500 / (1.1)5 = 310.46 10 years: PV = 500 / (1.1)10 = 192.77
4
Effects of Compounding
Simple interest Compound interest Consider the previous example
FV with simple interest = 1000 + 50 + 50 = 1100 FV with compound interest = 1102.50 The extra 2.50 comes from the interest of .05(50)
12
PV – One Period Example
Suppose you need $10,000 in one year for the down payment on a new car. If you can earn 7% annually, how much do you need to invest today?
8
Future Values – Example 3
Suppose you had a relative deposit $10 at 5.5% interest 200 years ago. How much would the investment be worth today?
FV = 10(1.055)200 = 447,189.84
16
英文版货币时间价值

Time value of money(货币的时间价值)1. Suppose you retire at age 65. You expect to live 20 more years and to spend $50,000 each year during your retirement. Assume an interest rate of 6 percent, how much money do you need to save by age 65 to support this expense plan?假定你65岁退休。
假话退休后再多活20年,在此期间每年花费$50,000。
假设利率为6%,那你需要在65岁之前存够多少钱来支持这份花费计划?50000*PVIFA6%,20=573500C. $573,4962. Which of the following amounts is the closest to the end value of investing $40,000 for 4 years at a continuously compounded rate of 15%? 40000*FVIF15%,4=69960下列哪个最接近$40,000的投资在连续复利率为15%下4年后的期末价值?B. $72,885Financial reporting and analysis(财务报告与分析)1. Please calculate net income based on the following information:请用下列信息计算净收益。
(300-180-30-40)*(1-34%)=33Sales = $300.00 销售收入= $300.00Cost of goods sold = $180.00 销货成本= $180.00Depreciation = $30.00 折旧= $30.00Interest paid = $40.00 利息支付= $40.00Tax rate = 34% 税率= 34%D. $33.002.Which of the following activities is/are in the category of use of cash:下列的哪种活动是现金使用的类别?I. increase in taxes payable. 应付税金的增加II. increase in accounts receivable. 应收账款的增加III. increase in prepayments. 预付账款的增加IV. dividend payments. 股利的支付A. Only IVUse the following data for questions 3-7Shown below are data taken from a recent annual report of Public Ltd. (Dollar in millions.)使用下列数据回答3-7题。
资金时间价值

资金时间价值1. 引言资金时间价值(Time Value of Money,简称TVM)是金融学中一个重要的概念,用于指出在不同时间点收到或支付的资金具有不同的价值。
在金融决策中,了解和计算资金时间价值对于评估不同投资机会的效益至关重要。
本文将介绍资金时间价值的基本概念、计算方法以及其在实际应用中的意义。
2. 基本概念2.1 现值现值(Present Value)是指未来收到或支付的一笔资金在当前时间点的价值。
现值可通过将未来的现金流量按照一定利率进行折现计算得出,反映了资金的时间价值。
现值可以用以下公式进行计算:PV = CF / (1 + r)^n其中,PV表示现值,CF表示未来的现金流量,r表示折现率,n表示未来现金流量的时间期。
2.2 未来值未来值(Future Value)是指当前时间点所投资的一笔资金在未来某个时间点所能够达到的价值。
未来值可通过将当前投资的资金按照一定利率进行复利计算得出,反映了资金的时间增值能力。
未来值可以用以下公式进行计算:FV = PV * (1 + r)^n其中,FV表示未来值,PV表示当前投资的现值,r表示复利率,n表示投资的时间期。
3. 计算方法3.1 单期计算单期计算是指将未来的一笔现金流量或当前投资的现值进行单独计算。
根据不同的情况,可以使用现值公式或未来值公式进行计算。
例如,如果要计算未来一年后一笔1000元的现金流量在当前时间点的现值,可以使用以下计算公式:PV = 1000 / (1 + r)^1如果要计算当前投资1000元在未来一年后的未来值,可以使用以下计算公式:FV = 1000 * (1 + r)^13.2 多期计算多期计算是指将未来的多笔现金流量或当前投资的现值进行连续计算。
在连续计算中,每个现金流量的现值或未来值都被计算进总体计算中。
计算多期时,可以使用如下公式进行计算:PV = CF1 / (1 + r)^1 + CF2 / (1 + r)^2 + ... + CFn / (1 + r)^nFV = PV1 * (1 + r)^1 + PV2 * (1 + r)^2 + ... + PVn * (1 + r)^n其中,CF1、CF2、…、CFn 表示未来现金流量,PV1、PV2、…、PVn 表示当前投资的现值。
资金时间价值的计算

资金时间价值的计算资金时间价值(Time Value of Money,简称TVM)是投资者在时间超过一年时对其资金的要求。
它的核心概念是认为资金的价值会随着时间的推移而发生变化,今天的一定金额的资金可能在未来的一些时间点上不再具有同样的价值。
了解和计算TVM对个人和企业在投资决策和财务规划中至关重要。
现值(Present Value,简称PV)是将未来的一笔金额折算为今天的价值。
在现值计算中,我们需要确定未来的金额、折现率和时间。
折现率是一个衡量资金时间价值的利率,它可以是固定利率也可以是不同期限的市场利率。
计算现值的公式为:PV=FV/(1+r)^n其中FV表示未来值,r表示折现率,n表示时间。
未来值(Future Value,简称FV)是今天一笔金额在未来其中一时间点上的价值。
计算未来值的公式为:FV=PV*(1+r)^n年金(Annuity)是一种定期支付或收取的金额,可以是等额本息还款、退休金或定期收入。
计算年金的公式有现值年金公式和未来值年金公式。
现值年金公式将未来一系列的付款或收款折算为今天的价值,计算公式如下:PV=PMT*[(1-(1+r)^(-n))/r]其中PMT表示每期支付或收取的金额,r表示折现率,n表示期数。
未来值年金公式则将未来一系列的付款或收款计算为未来其中一时间点上的价值,计算公式为:FV=PMT*[((1+r)^n-1)/r]利率(Rate)是资金增长或贬值的一个衡量标准。
在资金时间价值的计算中,我们可以通过已知现值、未来值和时间来计算利率。
计算利率的公式为:r=(FV/PV)^(1/n)-1其中FV表示未来值,PV表示现值,n表示时间。
通过现值、未来值、年金和利率的计算方法,我们可以根据不同的情况和需求来决策合适的投资策略、财务规划和借贷决策。
资金时间价值的概念和计算方法在个人理财、企业投资、贷款和退休规划等方面都具有广泛的应用。
了解和运用好这些方法可以帮助我们更好地管理和规划自己的财务。
Time value of money课后习题答案

2. a Present value of $5,000 today = $5,000 b. Present value of $15,000 received in 5 years at 9%: PV0 = $15,000(PVIF.09,5) = $15,000 (0.650) = $9,750 (calculator solution = $9,748.97) c. Present value of a 15 year, $1,000 annuity at 9%: PVAN0 = $1,000 (PVIFA.09,15) = $1,000(8.061) = $8,061 (calculator solution = $8,060.69) Therefore, you prefer $15,000 in five years because it has the highest present value.
CHAPTER 4 THE TIME VALUE The investment paying five percent compound interest is more attractive because you will receive interest not only on the principal amount each year, but interest will be earned on the previous year's interest as well. 2. The future value interest factor for 10 percent and two years is 1.210, whereas the present value interest factor for 10 percent and two years is 0.826. 3. As the interest rate increases, any annuity amount is being discounted by a higher value, thereby reducing the present value of the annuity. This can be seen in Table IV by looking across any row of successively higher interest rates. In contrast, the future value of an annuity increases as the interest (compounding) rate increases. (See Table III.) 4. Daily compounding is preferred because you will earn interest on the interest earned in the account each day. Table 4-6 illustrates this. 5. Annuity due computations are common for lease contracts and insurance policies, where payments are generally made at the beginning of each period. 6. As can be seen in Table 4-7, the more frequent the compounding period, the lower the present values. 7. a A marketing manager might use present value concepts to evaluate the success of an advertising or other promotional campaign, the benefits of which are likely to extend beyond one year in time. Also, a firm selling capital goods must be familiar with the type of present value economic analysis that customers will use to evaluate purchases.
补充资料:货币时间价值

3.单利现值的计算 p=F / (1+ i*n)
例2:你想5年后得到12000元钱,现在应一次存 入银行多少钱?年利率为4%,单利计息。
P= F/(1+ i*n)=12000/(1+4%*5)=10000(元)
(二)复利
每经过一个计息期,要将所生利息加入本金 再计利息,逐期滚算,俗称"利滚利"
1.复利终值的计算(已知P,求F) 复利终值也称为“本利和”,通常用F表 示。
注意:只经历一定时间,并不具有价值。如 将一笔钱放在家里保险柜中一定时间,不具 有价值。 把握: 1)货币经历一定时间 2)货币要经过投资和再投资过程,即投 入经营领域; 3)货币的时间价值是指增加的价值,是 剩余价值的一部分。
财务管理决策时两个重要的基本原则:
今天的一元钱比明天的一元钱更值钱。 保险的一元钱比有风险的一元钱更值钱。
(三)年金
年金指一定时期内每次等额收付的系列款项,记作 A
年金特点:(1)每期相隔时间相同(2)每期收入或 支出的金额相等如折旧、租金、利息、保险
金等。
年金的分类:普通年金、即付年金 递延年金、永续年金
1.普通年金(后付年金) 收支发生在每期期末的年金 (1)终值—一定时期内每期期末等额收付款项的复利终 值之和。
例4:你想5年后得到12000元钱,现在应一次存入 银行多少钱?年利率为4%,复利计息。 P= F×(1+i)-n =12000· (P/F,4%,5)=12000 × 0.8219 =9862.8(元)
例题:今天的1000元钱和十年后的2000元钱,你如何 选择? 已知:资金的机会成本是8%。 分析:不同时点上的资金价值不能比较, 故要折现到 同一时点 ① 利用终值比较:计算10年后1000元的价值与2000 元比较。 F=P(1+i)n F=1000(1+8%)10 =1000(2.1589)=¥2158.9 ② 利用现值比较:计算2000元现值与1000元比较。 P=F[1/(1+i)n] P=2000[1/(1+8%)10]=2000*(0.4632)=¥926.4
Chapter2 The Time Value Of Money

1) 某家长准备从其小孩的10岁生日起(包括10岁生日)到16岁生日止每年等额存入银行一笔钱,其目的是要在小孩的18、19、20、21四个生日每年从银行取出5000元,假设银行复利为8%,问该家长应在10岁至16岁生日每年等额存入银行多少钱?2)某工程建设时,三年内共贷款原值1800万元,其中第一年初用款500万元,第二年初用款700万元,第三年初用款600万元。
第五年末开始偿还,要求分5 年还清,问平均每年应还多少钱?(年利率为5%)3) 胜利公司股利预计从现在起按8%稳定增长(无期限),过去年度每股支付股利1.10元,投资者对于类似公司所期望的报酬为14%,请问:1)胜利公司的股票现在的价格应为多少?2)四年后该公司股票价格又为多少?4) 胜利公司股利预计第一年到第三年为1元/股,第四年开始按6%稳定增长(无期限),投资者对于类似公司所期望的报酬为10%,请问胜利公司的股票现在的价格应为多少?五年后该公司的股票价格又为多少?5)某公司的股票估计在3年内股利将高速增长,成长率为20%,在此以后转为正常增长,增长率为8%,公司最近支付的股利为1.5元每股。
假设公司要求的投资报酬率为15%,请估计该公司股票现在的价格。
(39.19)6)现有1200元,欲在19年后使其达到原来的3倍,选择投资机会时最低可接受的报酬率时多少?(6%)7)某人将1000元存入银行,存款年利率为8%,每季复利一次。
5年后此人可从银行取回多少钱?(1486)8)某公司拟购置一处房产,房主提出两种付款方案:①从现在起,每年年初支付20万元,连续支付10次,共200万元;②从第5年开始,每年年初支付25万元,连续支付10次,共250万元。
假设该公司的资金成本率(即要求的最低报酬率为10%,你认为该公司应选择哪个方案。
9)某企业拟购置一台柴油机,更新目前使用的汽油机,每月可节约燃料费用60元,但柴油机价格较汽油机高出1500元,问柴油机应使用多少年才合算(假设年利率为12%,每月复利一次)?(29个月)10)Finding the Dividend Torsion Corporation stock currently sells for $108 per share. The market requires a 15 percent return on the firm’s stock. If the company maintains a constant 7 percent growth rate in dividends, what was the most recent dividend per share paid on the stock?11)Negative Growth Ancient Items Co. is a mature manufacturing firm. The company just paid a $5 dividend, but management expects to reduce the payout by 9 percent per year, indefinitely. If you require a 12 percent return on this stock, what will you pay for a share today?12) Calculating the Number of Periods You expect to receive $40000 at graduation in two years. You plan on investing it at 5 percent until you have $60000. How long will you wait from now?13)You are looking into an investment that will pay you $1000 per year for the next 10 years. If you require a 10 percent return, what is the most you would pay for this investment?Multiple-Choice Quiz1. You want to buy an ordinary annuity that will pay you $4,000 a year for the next 20 years. You expect annual interest rates will be 8 percent over that time period. The maximum price you would be willing to pay for the annuity is closest to$32,000.$39,272.$40,000.$80,000.2. With continuous compounding at 10 percent for 30 years, the future value of an initial investment of $2,000 is closest to$34,898.$40,171.$164,500.$328,282.3. In 3 years you are to receive $5,000. If the interest rate were to suddenly increase, the present value of that future amount to you wouldfall.rise.remain unchanged.cannot be determined without more information.4. Assume that the interest rate is greater than zero. Which of the following cash-inflow streams should you prefer?Year1 Year2 Year3 Year4$400 $300 $200 $100$100 $200 $300 $400$250 $250 $250 $250Any of the above, since they each sum to $1,000.5. You are considering investing in a zero-coupon bond that sells for $250. At maturity in 16 years it will be redeemed for $1,000. What approximate annual rate of growth does this represent?8 percent.9 percent.12 percent.25 percent.6. To increase a given present value, the discount rate should be adjustedupward.downward.True.Fred.7. For $1,000 you can purchase a 5-year ordinary annuity that will pay you a yearly payment of $263.80 for 5 years. The compound annual interest rate implied by this arrangement is closest to8 percent.9 percent.10 percent.11 percent.8. You are considering borrowing $10,000 for 3 years at an annual interest rate of 6%. The loan agreement calls for 3 equal payments, to be paid at the end of each of the next 3 years. (Payments include both principal and interest.) The annual payment that will fully pay off (amortize) the loan is closest to$2,674.$2,890.$3,741.$4,020.9. When n = 1, this interest factor equals one for any positive rate of interest.PVIFFVIFPVIFAFVIFANone of the above (you can't fool me!)10. (1 + i)nPVIFFVIFPVIFAFVIFA。
货币的时间价值Time Value of Money

Time Value of Money ( 貨幣的時間價值 )0、今年的100元與明年的100元相比,何者的財富價值較高?答:今年的100元的財富價值較高,因為我如果將100元存放在台銀,一年後,以定存年利率1.5%計算,到期時我將擁有100*(1+1.5%)=101.5元>100元(明年);所以明年的100元與今年的100元在作財富價值比較時,須將明年的100元以適當的折現率( Appropriate Discounting Rate )折現成現值( Present Value ),這就是折現的觀念。
反之如果明年我欲擁有100元,華銀的一年定存利率同為1.5%,則我只須存入100/(1+1.5%)=98.522元,此98.522元就是明年100元的折現值(在折現率1.5%條件下)。
1、Compound Interest ( 複利 ) and Future Value( 未年值或終值 ))1(r C + 2)1(r C +nr C )1(+n r C FV )1(+= Future Value ( 終值 ) C=現金流量,r =利率(or 報酬率2、Future Value ( 終值,未來值 ))(,n r n FVIF PV FV =FVIF =Future Value Interest Factor( 終期利率因子 ),r = 利率,n=期數範例 1、小明存入東和銀行30,000元,年利率=6%,每年複利一次,5年後會變成多少錢?)(,n r n FVIF PV FV =78.146,40338226.1000,30%)61(000,3055=⨯=+⨯=FV而338226.15%,6=FVIF 0 1 2 n3、Present Value ( 現值 )在某一時點之金錢價值折現( Discounting )成目前的金錢價值。
)1(1r C+ )1(2r C+ )1(r nC+)1(r nCPV +=PV=Present Value( 現值 ),C=現金流量,r =折現率,n =期數)()1(,n r n nnPVIF FV r FV PV =+=PVIF r,n =Present Value Interest Factor(現值利率因子 )範例:小明希望存一筆錢在東和銀行,3年後能有100,000元,定存年利率為1.7%,則小明此時應存入多少錢才能達成目標?)1(r nCPV +=61.068,959506861.0000,100000,100%)7.11(3=⨯==+PV而 9506861.03%,7.1=PVIF0 1 2n4、Annuity ( 年金 )是指在某固定時間點的等額金額支付。
THE TIME VALUE OF MONEY
O VERVIEWA dollar in the hand today is worth more than a dollar to be received in the future because, if you had it now, you could invest that dollar and earn interest. Of all the techniques used in finance, none is more important than the concept of the time value of money,or discounted cash flow (DCF) analysis. The principles of time value analysis that are developed in this chapter have many applications, ranging from setting up schedules for paying off loans to decisions about whether to acquire new equipment.Future value and present value techniques can be applied to a single cash flow (lump sum), ordinary annuities, annuities due, and uneven cash flow streams. Future and present values can be calculated using a regular calculator or a calculator with financial functions. When compounding occurs more frequently than once a year, the effective rate of interest is greater than the quoted rate.The cash flow time line is one of the most important tools in time value of money analysis. Cash flow time lines help to visualize what is happening in a particular problem. Cash flows are placed directly below the tick marks, and interest rates are shown directly above the time line; unknown cash flows are indicated by question marks. Thus, to find the future value of $100 after 5 years at 5 percent interest, the following cash flow time line can be set up: Time: 0 1 2 3 4 5| | | | | | Cash flows: -100 FV5 = ?◆ A cash outflow is a payment, or disbursement, of cash for expenses, investments, and so on.◆ A cash inflow is a receipt of cash from an investment, an employer, or other sources.5%CHAPTER 3: THE TIME VALUE OF MONEY40Compounding is the process of determining the value of a cash flow or series of cash flows some time in the future when compound interest is applied. The future value is the amount to which a cash flow or series of cash flows will grow over a given period of time when compounded at a given interest rate. The future value can be calculated asFV n = PV(1 + k)n,where PV = present value, or beginning amount; k = interest rate per period; and n = number of periods involved in the analysis. This equation can be solved in one of two ways: numerically or with a financial calculator. For calculations, assume the following data that were presented in the time line above: present value (PV) = $100, interest rate (k) = 5%, and number of years (n) = 5.◆Compounded interest is interest earned on interest.◆To solve numerically, use a regular calculator to find 1 + k = 1.05 raised to the fifth power,which equals 1.2763. Multiply this figure by PV = $100 to get the final answer of FV5 = $127.63.◆With a financial calculator, the future value can be found by using the time value of moneyinput keys, where N = number of periods, I = interest rate per period, PV = present value, PMT = annuity payment, and FV = future value. By entering N = 5, I = 5, PV = -100, and PMT = 0, and then pressing the FV key, the answer 127.63 is displayed.♦Some financial calculators require that all cash flows be designated as either inflows or outflows, thus an outflow must be entered as a negative number (for example, PV= -100 instead of PV = 100).♦Some calculators require you to press a “Compute” key before pressing the FV key.◆ A graph of the compounding process shows how any sum grows over time at variousinterest rates. The greater the rate of interest, the faster is the rate of growth.♦The interest rate is, in fact, a growth rate.♦The time value concepts can be applied to anything that is growing.Finding the present value of a cash flow or series of cash flows is called discounting, and it is simply the reverse of compounding. In general, the present value is the value today of a future cash flow or series of cash flows. By solving for PV in the future value equation, the present value, or discounting, equation can be developed and written in several forms:CHAPTER 3: THE TIME VALUE OF MONEY41rate, one can utilize either of the two solution methods:♦Numerical solution: Divide $127.63 by 1.05 five times to get PV = $100.♦Financial calculator solution: Enter N = 5, I = 5, PMT = 0, and FV = 127.63, and then press the PV key to get PV = -100.◆The opportunity cost rate is the rate of return on the best available alternative investment ofequal risk.◆ A graph of the discounting process shows how the present value of any sum to be receivedin the future diminishes and approaches zero as the payment date is extended farther into the future. At relatively high interest rates, funds due in the future are worth very little today, and even at a relatively low discount rate, the present value of a sum due in the very distant future is quite small.The compounding and discounting processes are reciprocals, or inverses, of one another. In addition, there are four variables in the time value of money equations: PV, FV, k, and n. If three of the four variables are known, you can find the value of the fourth.◆If we are given PV, FV, and n, we can determine k by substituting the known values intoeither the present value or future value equations, and then solving for k. Thus, if you can buy a security at a price of $78.35 which will pay you $100 after 5 years, what is the interest rate earned on the investment?♦Numerical solution: Use a trial and error process to reach the 5% value for k. This is a tedious and inefficient process. Alternatively, you could use algebra to solve the timevalue equation.♦Financial calculator solution: Enter N = 5, PV = -78.35, PMT = 0, and FV = 100, then press the I key, and I = 5 is displayed.◆Likewise, if we are given PV, FV, and k, we can determine n by substituting the knownvalues into either the present value or future value equations, and then solving for n. Thus, if you can buy a security with a 5 percent interest rate at a price of $78.35 today, how long will it take for your investment to return $100?♦Numerical solution: Use a trial and error process to reach the value of 5 for n. This is a tedious and inefficient process. The equation can also be solved algebraically.♦Financial calculator solution: Enter I = 5, PV = -78.35, PMT = 0, and FV = 100, then press the N key, and N = 5 is displayed.An annuity is a series of equal payments made at fixed intervals for a specified number ofCHAPTER 3: THE TIME VALUE OF MONEY 42periods. If the payments occur at the end of each period, as they typically do, the annuity is an ordinary, or deferred, annuity. If the payments occur at the beginning of each period, it is called an annuity due. ◆ The future value of an ordinary annuity, FVA n , is the total amount one would have at theend of the annuity period if each payment were invested at a given interest rate and held to the end of the annuity period.♦ Defining FVA n as the future value of an ordinary annuity of n years, and PMT as the periodic payment, we can writethe FV key, and 315.25 is displayed.♦ For an annuity due, each payment is compounded for one additional period, so the future value of the entire annuity is equal to the future value of an ordinary annuity compounded for one additional period. Thus:FVA (DUE)n = PMT ⎥⎦⎤⎢⎣⎡+⨯⎭⎬⎫⎩⎨⎧-+)k 1(k 1)k 1(n .♦ Most financial calculators have a switch, or key, marked “DUE” or “BEG” that permitsyou to switch from end-of-period payments (an ordinary annuity) to beginning-of-period payments (an annuity due). Switch your calculator to “BEG” mode, and calculate as you would for an ordinary annuity. Do not forget to switch your calculator back to “END” mode when you are finished. ◆The present value of an ordinary annuity, PVA n , is the single (lump sum) payment today that would be equivalent to the annuity payments spread over the annuity period. It is the amount today that would permit withdrawals of an equal amount (PMT) at the end (or beginning for an annuity due) of each period for n periods.♦ Defining PVA n as the present value of an ordinary annuity of n years and PMT as the periodic payment, we can writePVA n = PMT ⎥⎦⎤⎢⎣⎡⎪⎪⎭⎫ ⎝⎛+∑=n 1t t )k 1(1 = PMT ⎥⎥⎥⎥⎦⎤⎢⎢⎢⎢⎣⎡+-k )k 1(11n = PMT ⎥⎦⎤⎢⎣⎡+--k )k 1(1n .CHAPTER 3: THE TIME VALUE OF MONEY43♦ Using a financial calculator, enter N = 3, I = 5, PMT = -100, and FV = 0, and then press the PV key, for an answer of $272.32.♦ One especially important application of the annuity concept relates to loans with constant payments, such as mortgages and auto loans. With these amortized loans the amount borrowed is the present value of an ordinary annuity, and the payments constitute the annuity stream. ◆The present value for an annuity due isPVA (DUE)n = PMT ⎥⎥⎥⎥⎦⎤⎢⎢⎢⎢⎣⎡+⨯⎪⎪⎭⎪⎪⎬⎫⎪⎪⎩⎪⎪⎨⎧+-)k 1(k )k 1(11n .♦ Using a financial calculator, switch to the “BEG” mode, and then enter N = 3, I = 5,PMT = -100, and FV = 0, and then press PV to get the answer, $285.94. Again, do not forget to switch your calculator back to “END” mode when you are finished.◆You can solve for the interest rate (rate of return) earned on an annuity.♦ To solve numerically, you must use the trial-and-error process and plug in different values for k in the annuity equation to solve for the interest rate.♦ You can use the financial calculator by entering the appropriate values for N, PMT, and either FV or PV, and then pressing I to solve for the interest rate.◆You can solve for the number of periods (N) in an annuity.♦ To solve numerically, you must use the trial-and-error process and plug in different values for N in the annuity equation to solve for the number of periods.♦ You can use the financial calculator by entering the appropriate values for I, PMT, and either FV or PV, and then pressing N to solve for the number of periods.A perpetuity is a stream of equal payments expected to continue forever. ◆ The present value of a perpetuity is:PVP =kPMTrate Interest Payment =.♦ For example, if the interest rate were 12 percent, a perpetuity of $1,000 a year wouldhave a present value of $1,000/0.12 = $8,333.33. ◆ A consol is a perpetual bond issued by the British government to consolidate past debts; in general, any perpetual bond.CHAPTER 3: THE TIME VALUE OF MONEY44◆The value of a perpetuity changes dramatically when interest rates change.Many financial decisions require the analysis of uneven, or nonconstant, cash flows rather than a stream of fixed payments such as an annuity. An uneven cash flow stream is a series of cash flows in which the amount varies from one period to the next.◆The term payment, PMT, designates constant cash flows, while the term CF designates cashflows in general, including uneven cash flows.◆The present value of an uneven cash flow stream is the sum of the PVs of the individualcash flows of the stream.♦The PV is found by applying the following general present value equation:PV = ∑=⎪⎪⎭⎫⎝⎛+n1 ttt)k1(1 CF.♦With a financial calculator, enter each cash flow (beginning with the t = 0 cash flow) into the cash flow register, CF j, enter the appropriate interest rate, and then press theNPV key to obtain the PV of the cash flow stream.♦Be sure to clear the cash flow register before starting a new problem.◆Similarly, the future value of an uneven cash flow stream, or terminal value, is the sum ofthe FVs of the individual cash flows of the stream.♦The FV can be found by applying the following general future value equation:FV n = ∑=-+n 1 ttnt)k1(CF.♦Some calculators have a net future value (NFV) key which allows you to obtain the FV of an uneven cash flow stream.◆We generally are more interested in the present value of an asset’s cash flow stream than inthe future value because the prese nt value represents today’s value, which we can compare with the price of the asset.♦Once we know its present value, we can find the future value of an uneven cash flow stream by treating the present value as a lump sum amount and compounding it to thefuture period.◆If one knows the relevant cash flows, the effective interest rate can be calculated efficientlywith a financial calculator. Enter each cash flow (beginning with the t = 0 cash flow) into the cash flow register, CF j, and then press the IRR key to obtain the interest rate of anCHAPTER 3: THE TIME VALUE OF MONEY45uneven cash flow stream.♦ IRR stands for internal rate of return , which is the return on an investment.Annual compounding is the arithmetic process of determining the final value of a cash flow or series of cash flows when interest is added once a year. Semiannual, quarterly, and other compounding periods more frequent than on an annual basis are often used in financial transactions. Compounding on a nonannual basis requires an adjustment to both the compounding and discounting procedures discussed previously. Moreover, when comparing securities with different compounding periods, they need to be put on a common basis. This requires distinguishing between the simple, or quoted, interest rate and the effective annual rate. ◆ The simple , or quoted, interest rate is the contracted, or quoted, interest rate that is used tocalculate the interest paid per period. ◆ The periodic rate is the interest rate charged per period.Periodic rate = Stated annual interest rate/Number of periods per year.◆ The annual percentage rate, APR, is the periodic rate times the number of periods per year. ◆The effective annual rate, EAR, is the rate that would have produced the final compounded value under annual compounding. The effective annual rate is given by the following formula:Effective annual rate (EAR) = ,0.1m k 1mSIMPLE -⎪⎭⎫⎝⎛+where k SIMPLE is the simple, or quoted, interest rate (that is, the APR), and m is the numberof compounding periods (interest payments) per year. The EAR is useful in comparing securities with different compounding periods. ◆For example, to find the effective annual rate if the simple rate is 6 percent and semiannual compounding is used, we have:EAR = (1 + 0.06/2)2 – 1.0 = 6.09%.◆For annual compounding use the formula to find the future value of a single payment (lump sum):FV n = PV(1 + k)n .CHAPTER 3: THE TIME VALUE OF MONEY 46♦ When compounding occurs more frequently than once a year, use this formula:FV n = PV nm SIMPLE m k 1⨯⎪⎭⎫⎝⎛+.Here m is the number of times per year compounding occurs, and n is the number of years.◆The amount to which $1,000 will grow after 5 years if quarterly compounding is applied to a nominal 8 percent interest rate is found as follows:FV n = $1,000(1 + 0.08/4)(4)(5) = $1,000(1.02)20 = $1,485.95.♦ Financial calculator solution: Enter N = 20, I = 2, PV = -1000, and PMT = 0, and then press the FV key to find FV = $1,485.95.◆The present value of a 5-year future investment equal to $1,485.95, with an 8 percent nominal interest rate, compounded quarterly, is found as follows:.000,1$)02.1(95.485,1$PV /4)08.01(PV 95.485,1$20(4)(5)==+=♦ Financial calculator solution: Enter N = 20, I = 2, PMT = 0, and FV = 1485.95, and then press the PV key to find PV = -$1,000.00.◆In general, nonannual compounding can be handled one of two ways.♦ State everything on a periodic rather than on an annual basis. Thus, n = 6 periods rather than n = 3 years and k = 3% instead of k = 6% with semiannual compounding.♦ Find the effective annual rate (EAR) with the equation below and then use the EAR as the rate over the given number of years.EAR = .0.1m k 1mSIMPLE -⎪⎭⎫⎝⎛+An important application of compound interest involves amortized loans, which are paid offin equal installments over the life of the loan.◆The amount of each payment, PMT, is found using a financial calculator by entering N (number of years), I (interest rate), PV (amount borrowed), and FV = 0, and then pressing the PMT key to find the periodic payment.◆ Each payment consists partly of interest and partly of repayment of the amount borrowed (principal). This breakdown is often developed in a loan amortization schedule .CHAPTER 3: THE TIME VALUE OF MONEY47♦ The interest component is largest in the first period, and it declines over the life of the loan as the outstanding balance of the loan decreases.♦ The repayment of principal is smallest in the first period, and it increases thereafter.The text discussion has involved three different interest rates. It is important to understand their differences.◆The simple , or quoted, rate, k SIMPLE , is the interest rate quoted by borrowers and lenders. This quotation must include the number of compounding periods per year.♦ This rate is never shown on a time line, and it is never used as an input in a financial calculator unless compounding occurs only once a year. ♦ k SIMPLE = Periodic rate ⨯ m = Annual percentage rate = APR.◆The periodic rate, k PER , is the rate charged by a lender or paid by a borrower each interest period. Periodic rate = k PER = k SIMPLE /m.♦ The periodic rate is used for calculations in problems where two conditions hold: (1) payments occur on a regular basis more frequently than once a year, and (2) a payment is made on each compounding (or discounting) date.♦ The APR, or annual percentage rate, represents the periodic rate stated on an annual basis without considering interest compounding. The APR never is used in actual calculations; it is simply reported to borrowers. ◆The effective annual rate, EAR, is the rate with which, under annual compounding, we would obtain the same result as if we had used a given periodic rate with m compounding periods per year.♦ EAR is found as follows:EAR = .0.1m k 1mSIMPLE -⎪⎭⎫⎝⎛+In Appendix 3A we discuss using spreadsheets to solve time value of money problems.In Appendix 3B we discuss using interest tables to solve time value of money problems.In Appendix 3C we discuss how to generate a loan amortization schedule using a financial calculator.S ELF-TEST Q UESTIONSDefinitionalCHAPTER 3: THE TIME VALUE OF MONEY481.A(n) ______ _________ is a payment, or disbursement, of cash for expenses, investments,and so on.2.A(n) ______ ________ is a receipt of cash from an investment, an employer, or othersources.3._____________ is the process of determining the value of a cash flow or series of cashflows some time in the future.4.The ________ _______ is the amount to which a cash flow or series of cash flows willgrow over a given period of time when compounded at a given interest rate.5.The beginning value of an account or investment in a project is known as its ________________.ing a savings account as an example, the difference between the account’s present valueand its future value at the end of the period is due to __________ earned during the period.7.The expression PV(1 + k)n determines the ________ _______ of a sum at the end of ___periods.8.Finding the present value of a cash flow or series of cash flows is often referred to as_____________, and it is simply the reverse of the _____________ process.9.The _____________ ______ ______ is the rate of return on the best alternative investmentof equal risk.10. A series of equal payments at fixed intervals for a specified number of periods is a(n)_________. If the payments occur at the end of each period it is a(n) __________ annuity, while if the payments occur at the beginning of each period it is an annuity _____.11.A(n) ____________ is a stream of equal payments expected to continue forever.12.The term PMT designates __________ cash flows, while the term CF designates cash flowsin general, including ________ cash flows.13.The present value of an uneven cash flow stream is the _____ of the PVs of the individualcash flows of the stream.49 14.Since different types of investments use different compounding periods, it is important todistinguish between the quoted, or ________, interest rate and the ___________ annual interest rate, the rate that would have produced the final compound value under annual compounding.15.____________ time periods are used when payments occur within periods, instead of ateither the beginning or the end of periods.16.___________ loans are paid off in equal installments over their lifetime and are animportant application of compound interest.17.The __________ rate is equal to the simple interest rate divided by the number ofcompounding periods per year.Conceptual18.If a bank uses quarterly compounding for savings accounts, the simple interest rate will begreater than the effective annual rate (EAR).a. Trueb. False19.If money has time value (that is, k > 0), the future value of some amount of money willalways be more than the amount invested. The present value of some amount to be received in the future is always less than the amount to be received.a. Trueb. False20.You have determined the profitability of a planned project by finding the present value ofall the cash flows from that project. Which of the following would cause the project to look less appealing, that is, have a lower present value?a.The discount rate decreases.b.The cash flows are received in later years (further into the future).c.The discount rate increases.d.Statements b and c are correct.e.Statements a and b are correct.21.As the discount rate increases without limit, the present value of a future cash inflowa.Gets larger without limit.b.Stays unchanged.50c.Approaches zero.d.Gets smaller without limit; that is, approaches minus infinity.e.Goes to e k n.5122.Which of the following statements is correct?a.Except in situations where compounding occurs annually, the periodic interest rateexceeds the simple interest rate.b.The effective annual rate always exceeds the simple interest rate, no matter how few ormany compounding periods occur each year.c.If compounding occurs more frequently than once a year, and if payments are made attimes other than at the end of compounding periods, it is impossible to determinepresent or future values, even with a financial calculator. The reason is that under theseconditions, the basic assumptions of discounted cash flow analysis are not met.d.Assume that compounding occurs quarterly, that the simple interest rate is 8 percent,and that you need to find the present value of $1,000 due 10 months from today. Youcould get the correct answer by discounting the $1,000 at 8.2432 percent for 10/12ths ofa year.e.Statements a, b, c, and d are all false.S ELF-TEST P ROBLEMS(Note: In working these problems, you may get an answer which differs from ours by a few cents due to differences in rounding. This should not concern you; just choose the closest answer.)1.Assume that you purchase a 6-year, 8 percent savings certificate for $1,000. If interest iscompounded annually, what will be the value of the certificate when it matures?a.$630.17b. $1,469.33c. $1,677.10d. $1,586.87e. $1,766.332. A savings certificate similar to the one in the previous problem is available with theexception that interest is compounded semiannually. What is the difference between the ending value of the savings certificate compounded semiannually and the one compounded annually?a.The semiannual certificate is worth $14.16 more than the annual certificate.b.The semiannual certificate is worth $14.16 less than the annual certificate.c.The semiannual certificate is worth $21.54 more than the annual certificate.d.The semiannual certificate is worth $21.54 less than the annual certificate.e.The semiannual certificate is worth the same as the annual certificate.523. A friend promises to pay you $600 two years from now if you loan him $500 today. Whatannual interest rate is your friend offering?a. 7.55%b. 8.50%c. 9.54%d. 10.75%e. 11.25%4.At an inflation rate of 9 percent, the purchasing power of $1 would be cut in half in just over 8years (some calculators round to 9 years). How long, to the nearest year, would it take for the purchasing power of $1 to be cut in half if the inflation rate were only 4 percent?a. 12 yearsb. 15 yearsc. 18 yearsd. 20 yearse. 23 years5.You are offered an investment opportunity with the “guarantee” that your investment willdouble in 5 years. Assuming annual compounding, what annual rate of return would this investment provide?a. 40.00%b. 100.00%c. 14.87%d. 20.00%e. 18.74%6.You decide to begin saving toward the purchase of a new car in 5 years. If you put $1,000at the end of each of the next 5 years in a savings account paying 6 percent compounded annually, how much will you accumulate after 5 years?a. $6,691.13b. $5,637.09c. $1,338.23d. $5,975.32e. $5,731.947.Refer to Self-Test Problem 6. What would be the ending amount if the payments weremade at the beginning of each year?a. $6,691.13b. $5,637.09c.$1,338.23d. $5,975.32e. $5,731.948.Refer to Self-Test Problem 6. What would be the ending amount if $500 payments weremade at the end of each 6-month period for 5 years and the account paid 6 percent compounded semiannually?a. $6,691.13b.$5,637.09c. $1,338.23d. $5,975.32e. $5,731.949.Calculate the present value of $1,000 to be received at the end of 8 years. Assume aninterest rate of 7 percent.a. $582.01b. $1,718.19c. $531.82d. $5,971.30e. $649.3753 10.Jane Smith has $20,000 in a brokerage account, and she plans to contribute an additional$7,500 to the account at the end of every year. The brokerage account has an expected annual return of 8 percent. If Jane’s goal is to a ccumulate $375,000 in the account, how many years will it take for Jane to reach her goal?a. 5.20b. 10.00c. 12.50d. 16.33e. 18.4011. How much would you be willing to pay today for an investment that would return $800each year at the end of each of the next 6 years? Assume a discount rate of 5 percent.a. $5,441.53b. $4,800.00c. $3,369.89d. $4,060.55e. $4,632.3712.You have applied for a mortgage of $60,000 to finance the purchase of a new home. Thebank will require you to make annual payments of $7,047.55 at the end of each of the next20 years. Determine the interest rate in effect on this mortgage.a. 8.0%b. 9.8%c. 10.0%d. 5.1%e. 11.2%13.If you would like to accumulate $7,500 over the next 5 years, how much must you depositeach six months, starting six months from now, given a 6 percent interest rate and semiannual compounding?a. $1,330.47b. $879.23c. $654.23d. $569.00e. $732.6714. A company is offering bonds that pay $100 per year indefinitely. If you require a 12 percentreturn on these bonds (that is, the discount rate is 12 percent), what is the value of each bond?a. $1,000.00b. $962.00c. $904.67d. $866.67e.$833.3315.What is the present value (t = 0) of the following cash flows if the discount rate is 12percent?0 1 2 3 4 512%| | | | | |0 2,000 2,000 2,000 3,000 -4,000a.$4,782.43b. $4,440.51c. $4,221.79d. $4,041.23e. $3,997.9816.What is the effective annual percentage rate (EAR) of 12 percent compounded monthly?。
Lecture 2 The time value of money

Time value
How
much one dollar today can earn during the year,in a economic activity?
interest interest rate
Composing of interest rate
With
a Compound interest i, which of the followings would prefer?
$C at 0; $C (1+i)n at n.
Remarks
at 0 is equivalent to $ C (1+i)n at n; Or $C/ (1+i)n at 0 is equivalent to $ C at n; or $1/ (1+i)n at 0 is equivalent to $ 1 at n.
What is An?
Simple interest
? years
1 2 …… n
Capital + interest
A1=C+Ci =C(1+i) A2=C+2Ci =C(1+2i) …… An=C+nCi =C(1+ni)
interest
Ci 2Ci …… nCi
The future value of $1 today is linear to the length of time under simple interest rate
Simple interest: simple interest can not earn further interest.
时间价值英语作文

时间价值英语作文Time Value of Money.Time is money. This phrase is often used to emphasize the importance of time in our lives. However, when it comes to finance, time can actually be worth money. This is because of the concept of time value of money.The time value of money is the idea that money received or paid out at different times has different values. Thisis because of the potential to earn interest or returns on the money over time. For example, $100 received today is worth more than $100 received a year from now because the $100 received today can be invested and earn interest over the next year.The time value of money is an important concept in finance because it helps individuals and businesses make better financial decisions. By understanding the time value of money, they can calculate the present value of futurecash flows, determine the best investment options, and make informed decisions about borrowing and lending money.One of the most common ways to calculate the time value of money is through the use of the present value formula. This formula takes into account the amount of money, the interest rate, and the time period to calculate the present value of future cash flows. By comparing the present value of different cash flows, individuals and businesses can determine which option is the most financially beneficial.In addition to the present value formula, there are other financial tools and concepts that are related to the time value of money. These include compound interest, annuities, and the internal rate of return. By understanding these concepts, individuals and businesses can make more informed financial decisions and maximize their returns.In conclusion, the time value of money is an important concept in finance that helps individuals and businesses make better financial decisions. By understanding thepotential value of money over time, they can make informed decisions about investing, borrowing, and lending money.。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
Yields
• The higher the yield, the higher the risk. • The lower the yield, the lower the risk.
Money doesn’t grow on trees…
But money does grow…
They say…
Valuation Mathematics
• Practically every calculation made by a valuer involves compound interest theory…valuation tables are a series of compound interest tables with what can be described as variations around the basic compound interest theme.
Why Investment?
Economic Wealth
• Under neoclassical economic theory, the 3 main factors that contribute to economic wealth are normally taken to be:
People Money Land
– the provision of shops, offices for corporate use and private leisure facilities
• This is on the basis of economic demand, as expressed through the supply and demand.
Eg. A one off investment for a fixed time period.
The table looks like this…
• Whenever we are holding a sum of money that is or could be earning a return.
The Amount of £1 Table
• Calculates the amount to which £1 will grow in a given number of years at varying rates of interest • Is based on the simple formula £1 * (1+i)^n where i is the interest rate and n is the number of years • The table assumes that interest is payable yearly in arrears
Sayce (2006) pg.1
Field / Yield
Yield - Return
Yields
• Issac (1998:19) lists the components of yields as follows:
– Compensation for the time preference of money – An inflation allowance to maintain the real value of the return – An element for risk-taking
• These decisions are generally taken on the basis of least cost and value for money. Sayce (2006) pg.1
Private Individuals & Corporate Bodies
• Where the allocation decisions are based on demand for example:
How does money grow?
Compounding
/process/10_costs/costs_01_discounting.htm
Compounding
• If we want to know the future worth of money that we have now … • We need to make an allowance for time • We do this by adding the interest that the original sum will earn together with interest on the interest that will be earned • The process of calculating that future worth is called “Compounding” or sometimes “accumulating”
Amt £1
• The Amount of £1
Amt £1 = (1 + i)^n
Why?
• This table shows the amount to which a fixed sum will accumulate to if it earns a fixed rate of compound interest for a fixed number of years.
Sayce (2006) pg.1
• Each of these resources people, money and land can be argued to be in fixed supply.
Economic Theory
• Each resource has a value to both the individual and society. • In Britain there is a mixed economy. • The government base their resource allocation decisions on basis of need. • Private individuals and corporate bodies base their resource allocation decisions on basis of economic demand.
Suzhou University of Science and Technology, China & University of Glamorgan, UK
Partnership Visit April 2011
Construction Economics – The Time Value of Money
When to use the Amount of table
• Example. To what sum will £100 in my bank account grow if the interest rate is 3.75% and I leave it there for 5 years (Exclude tax issues)
Construction Economics
The Time Value of Money
Introduction
• When undertaking property valuations and costing construction contracts it is important to: – firstly to understand the value of money. – secondly know how it is calculated. • We also need to understand investment and why people invest.
How do we calculate future worth?
• We can use Parry’s Valuation Tables • Or we could use a calculator • Or we could enter the data directly into a spreadsheet
(Millington 4th Ed. p.98)
© Shane P Galvin (BE1S71 - Lecture 3)
Valuation Mathematics
• It may be easy to calculate and apply valuation mathematics but it is important to understand the processes and the reasoning behind the decisions to use the various methods.
Government
• Where Real Estate allocation decisions are based on need for example:
– the provision of public goods and services such as hospital and schools
Why does money grow?
• • • • Time preference Compensatory principle A risk premium Inflation
• So money grows at an interest rate or yield.
Time
• Payments are at different times. • Costs are paid at different times. • If time is money then the value of money changes at different times. • It changes at a market rate or comparable rate to an alternative investment.
