高级微观经济学 讲义5
微观经济学课件第5章及应用

国际政治风险
国际政治局势的不稳定可能导致 贸易中断、制裁等问题,对企业
经营带来不确定性。
05
微观经济学第5章前沿研究动态
不完全竞争市场理论新发展
不完全竞争市场的新模型
针对传统模型的缺陷,新的模型考虑了更多现实因素,如信息不 对称、市场进入壁垒等。
不完全竞争与经济增长
研究发现,不完全竞争市场在一定程度上可以促进技术创新和经济 增长,但过度的垄断会抑制竞争和创新。
指市场中存在一定程度的市场势力,使得价格和 产量不能完全由市场供求关系决定的市场类型。
不完全竞争市场的原因
包括产品差异、市场进入壁垒、信息不对称等因 素。
3
不完全竞争市场的类型
主要包括垄断市场、寡头市场和垄断竞争市场。
垄断市场特征与形成原因
垄断市场的特征
市场上只有一个卖者,且该卖者拥有控制价格和产量的市场势力 。
行为经济学与微观经济学的融合
行为经济学与微观经济学的融合可以为不完全竞争市场的研究提供新的视角和方法论支持。
实验方法在微观经济学中应用前景
01
实验方法在微观经济 学中的优势
实验方法可以控制实验条件和环境因 素,从而更准确地揭示经济现象和规 律。
02
实验方法在微观经济 学中的应用案例
近年来,越来越多的学者开始运用实 验方法来研究微观经济学领域的问题 ,如市场机制设计、拍卖理论等。
微软公司反垄断案例解读
微软反垄断案的背景
微软公司在操作系统和办公软件市场占据主导地位,涉嫌利用市场优势地位进行不正当 竞争和限制竞争行为。
反垄断调查和诉讼过程
多国政府对微软展开反垄断调查和诉讼,指控其滥用市场支配地位,妨碍创新和市场竞 争。
微观经济学第五章.pptx

C
TC
VC
M
FC
O
Q
短期总成本曲线
二、单位成本分析
⒈短期平均成本 平均成本:生产每一单位产量平均所支付的费用。与
上述三种总成本函数相对应,存在三种短期平均成本: ①平均固定成本AFC等于固定成本TFC除以产量: AFC=FC/Q ②平均可变成本AVC等于可变成本TVC除以产量: AVC=VC/Q ③平均总成本ATC等于总成本STC除以产量: AC=TC/Q =AFC+AVC
C=g (Q) 由于理论上将厂商投入和产出的转换过程分为短期和 长期,因此,成本函数也分为短期成本函数和长期成本函 数。短期指某些生产要素的使用量(通常指资本设备)固 定不变的时期。在长期,所有的生产要素都是可变的,不 存在固定不变的成本。
第二节 短期成本
一、短期总成本
短期总成本:厂商在短期生产中所付出的全部成本, 包括固定成本和可变成本两部分。
LTC是STC的包络曲线 LTC曲线从原点出发向右上方倾斜 LTC曲线是扩展线的转换形态
LTC是STC的包络曲线
不同的短期总成本曲线STC表示不同的生产规模
(FC不同) 。
LTC
C
STC4
STC1 STC2 STC3
FC4
FC3 FC2 FC1
O Q1 Q2
LTC曲线从原点出发 向右上方倾斜
Q Q3
成本。 例如,对于一个将废物排放到附近河流中
去的钢厂来说,其处理废物的私人成本只不过 是把废物排放河中所需支付的费用。但别的厂 商或消费者要使用具有一定纯净度的河水,就 必须额外支付使河水净化所需的费用,排放废 物的社会成本就大于私人成本。
二、成本函数
成本函数表示成本与产量之间的关系。用C表示成本, Q表示产量,成本函数可写为:
《高级微观经济学》课件

公共支出
政府通过提供公共服务和基础 设施,弥补市场失灵,提高社 会福利。
监管和行政干预
政府对市场进行监管和行政干 预,防止垄断和不公平竞争。
市场失灵与政府干预的案例分析
环境污染案例
政府通过制定环保法规和排污标准,限制企 业排污,保护环境。
医疗保障案例
政府通过提供医疗保险和医疗救助,弥补市 场失灵,保障公民健康。
最优消费选择
在预算约束下,消费者选择能够最大化效用的商品组合。
边际替代效应
描述消费者在保持效用不变的情况下,一种商品对另一种商品的 替代程度。
消费者行为理论的扩展
风险偏好与不确定性
研究消费者在面临风险和不确定性时的消费行 为。
跨期消费选择
探讨消费者在不同时期之间的消费决策和储蓄 行为。
消费外部性
分析消费行为对其他个体或社会的影响,以及如何通过政策干预来改善消费行 为。
微观经济学的重要性
微观经济学是现代经济学的重要组成部分,它为政策制定者、企业家和消费者提供了理解和预测市场运作的基础 。通过研究微观经济学,人们可以更好地理解市场机制、价格体系和资源配置,从而做出更明智的决策。
微观经济学的基本假设和概念
基本假设
微观经济学通常基于一些基本假设, 如完全竞争、理性行为、完全信息等 。这些假设为理论分析提供了基础, 但在实际生活中可能并不完全成立。
公共选择理论与政治经济学
01
公共选择理论
研究公共物品和服务的供给和需求,以及政府决策的经济学分析。
02
政治经济学
研究政治和经济之间的相互作用,以及政治制度对经济发展的影响。
03
总结
公共选择理论和政治经济学是微观经济学的前沿领域,它们对于理解政
微观经济学讲义

微观经济学讲义第一节西方经济学概论一、稀缺性1.相对于人类社会的无穷欲望而言,经济物品,或者说生产这些物品所需要的资源总是不足的。
这种资源的相对有限性就是稀缺性。
2.稀缺性的相对性是指相对于无限的欲望而言,再多的资源也是稀缺的。
3.稀缺性的绝对性是指它存在于人类历史的各个时期和一切社会。
稀缺性是人类社会永恒的问题,只要有人类社会,就会有稀缺性。
4.经济学产生于稀缺性的存在。
5.稀缺性的存在决定了一个社会和个人必须作出选择。
二、选择1.稀缺性的存在决定了一个社会和个人必须作出选择。
2.选择就是用有限的资源去满足什么欲望的决策。
它包括“生产什么”、“如何生产”和“为谁生产”三个问题。
这三个问题被称为资源配置问题。
三、机会成本1.经济学是研究选择的,要选择就要有所舍弃,舍弃的东西就是机会成本。
2.机会成本并不是实际上的支出,而是一种观念上的支出。
四、微观经济学与宏观经济学微观经济学以单个经济单位为研究对象,通过研究单个经济单位的经济行为和相应的经济变量单项数值的决定,来说明价格机制如何解决社会的资源配置问题。
宏观经济学以整个国民经济为研究对象,通过研究经济中各有关总量的决定及其变化,来说明资源如何才能得到充分利用。
微观经济学与宏观经济学的区别:第一,研究的对象不同。
微观经济学的研究对象是单个经济单位的经济行为,宏观经济学的研究对象是整个经济。
第二,解决的问题不同。
微观经济学解决的问题是资源配置,宏观经济学解决的问题是资源利用。
第三,中心理论不同。
微观经济学的中心理论是价格理论,宏观经济学的中心理论是国民收入决定理论。
第四,研究方法不同。
微观经济学的研究方法是个量分析,宏观经济学的研究方法是总量分析。
微观经济学与宏观经济学的联系:第一,微观经济学与宏观经济学是互相补充的。
第二,微观经济学与宏观经济学的研究方法都是实证分析。
第三,微观经济学是宏观经济学的基础。
五、理论(一)理论的内容一个完整的理论包括定义、假设、假说和预测。
微观经济学第五章PPT课件

2020/3/27
2
第五章 成本论
教学内容: 第一节 成本的概念 第二节 短期总产量和短期总成本 第三节 短期成本曲线 第四节 长期成本曲线
2020/3/27
3
第五章 第一节 成本的概念
2020/3/27
4
第五章 第一节 成本的概念
一、机会成本 机会成本是指将一定的资源用于某项特
定用途时,所放弃的该项资源用于其他用 途时所能获得的最大收益。
2020/3/27
10
第五章 第一节 成本的概念
门脸房是出租还是自己经营?
假如你家有一个门脸房,你用它开了一 家杂货店。一年下来,你算账的结果是得 到5万元人民币的盈利。如果把门脸房出租, 按市场价一年是2万元,又假定你原来有工 作,年收入是3万元。那么,你认为门脸房 是出租还是自营好?
答案:出租比自营好?
微观经济学
2020/3/27
1
第五章 成本论
引言: 成本本章分以析生是产以函生数产的函变数动的规分律析为为基基础础,进从
行要的素,投因入而的成价本值分形析态也(即分成短本期)角和度长详期细两地种。 考在察成厂本商分的析生中产,成经本济与学产家量仍的然关假系设,要即素成 价本格函不数变。,此从外而,把还成要本讨仅论仅在表长述期为中是,产成量本 的和函规数模。之间的关系。
2020/3/27
6
第五章 第一节 成本的概念
二、显成本和隐成本
生产成本=显成本+隐成本
显成本:即会计成本,是厂商在要素市场 上购买或租用所需要的生产要素的实际支出 (帐面上看得见的成本)。
隐成本:厂商自己所拥有的且被用于该企 业生产过程的那些生产要素的总价值(自己 应该得到的报酬,帐面上没反映的成本)。
高级微观经济学讲义

高级微观经济学讲义:均衡、福利与寻租理论主讲人:邢祖礼西南财经大学经济学院(2013秋季)一、教学目的与要求通过本讲,让学生了解局部均衡、一般均衡的基本思想,掌握帕累托最优、超额需求函数、经济核等重要概念,熟悉福利经济学第一定理、第二定理、核定理,能够较为详细的理解均衡的存在性问题。
二、基本内容与课时安排1、局部均衡(3课时)2、交换均衡:求解(3课时)3、生产均衡(3课时)4、寻租与中国经济增长的特征(3课时)共计:12课时(2周)三、参考书目杰弗瑞.杰里菲利普.瑞尼:《高级微观经济学》,上海财经大学出版社2002年。
Andreu Mas-Colell Michael D.Whinston and Jerry R.Green:“Microeconomic Theory”,上海财经大学出版社2005年。
附:讲义的基本内容高级微观经济学讲义:均衡、福利与寻租理论邢祖礼西南财经大学经济学院2013年秋季第一讲:局部均衡分析一、竞争性均衡1、拟线性效用函数Quasi-linear utility function:)(),(i i i i i i x m x m u ϕ+=, i x 是一个消费产品, i m 是其他所产品的支出。
这种函数形式暗含两个假设:(1) x 产品没有收入效应,即x 产品的边际效用独立于收入m ;(2) x 产品的价格不影响其他产品的价格。
通过这两个假设,我们可以得出:其他产品的价格独立于x 产品。
2、需求:)(max i i i x m ϕ+s.t. i i i m p x y +⋅≤ (*)从 (*)中, 我们有:i i i m y p x =-⋅代入目标函数有:max ()ii i i i x x p x y φ-⋅+*()i i x p ϕ'=。
需求量 *i x 依赖于 p 并随着 p 变化. *i x 独立于收入;市场需求∑==Ii i p x p X 1*)()(, 它独立于禀赋分配和产权。
微观经济学第5章课件

O
Q1
Q2
Q3
Q
5.3 长期成本
5.3.2 LAC曲线
LTC LAC= Q
LAC曲线是无数条SAC曲线的包络线,LAC曲线呈U型
5.3.3、LMC曲线
LMC=
dLTC dQ
LMC是LTC曲线上任一点切线的斜率。
5.3 长期成本
5.3.4 最优工厂与最优产量
1、最优产量:optimum plant 在短期内厂商只能利用现有规模进行生产,在此规模 下,短期平均成本最低时的产量,称为这一规模的最优产 量。 2、最优工厂 在长期中,厂商根据市场预测来确定产量,为生产这 一产量可以任意调节一切要素投入,建立一个规模适当的 工厂,以求这一产量下的总成本最小,这一最适当的工厂 规模称为这一产量下的最优工厂。
递增的速度
H
递减的速度
FC
AFC
Q0
图1
概念:
①SMC曲线是VC曲线上任一点的斜 率和相应的产量构成的点的连 线。
短期成本曲线之间的关系
C SMC
◆结论:
①AVC曲线呈U型 ②SMC曲线呈U型
SAC ③SMC与SAC、AVC最低点相交
D
AVC
④为什么D点晚于E点?
E
Q
图2
因为,SAC=AVC+AFC 当AFC曲线的下降趋势大于AVC 曲线的上升趋势时,SAC曲线表 现为下降趋势。 当AFC曲线的上升趋势小于ACV 曲线的下降趋势时,SAC曲线表 现为上升趋势。 所以,SAC与AVC最低点出现不 一致,AVC出现早,SAC出现晚。
厂商每增加一个 单位产品的生产 (产量),而引 起的总成本的变 化量
5.2 短期成本
5.2.2、生产函数与成本函数的对偶性
高级微观经济学课件上海财经大学夏纪军

拍卖理论
拍卖的定义:一种通过公开竞价的方式,将物品或服务卖给出价最高 的人的交易方式 拍卖的类型:包括英式拍卖、荷兰式拍卖、第一价格密封拍卖、第 二价格密封拍卖等
拍卖的机制:包括价格机制、信息机制、策略机制等
拍卖的应用:在商品交易、资源配置、公共决策等领域有广泛应用
不确定性下的决策
决策理论:描述不确定性下的 决策过程和结果
信息经济学:研究信息不对称对经 济行为的影响
公共选择理论:研究公共决策的经 济学原理和方法
高级微观经济学与初级微观经济学的区别
研究对象:高级微观经济学主要研究消费者、生产者、市场等微观经济主体的行为和决策,而初 级微观经济学则主要研究价格、供给、需求等宏观经济现象。
理论深度:高级微观经济学的理论深度较初级微观经济学更深,涉及到更多的数学模型和理论推 导。
应用领域:市场营销、人力 资源管理、公共政策等
高级微观经济学的学习方 法
章节副标题
学习高级微观经济学的目的和方法
目的:掌握微观经济学的基本原理和理 论,提高分析和解决问题的能力
方法:做习题,巩固和运用所学知识
方法:阅读教材,理解基本概念和理 论
方法:参加讨论,交流观点和经验
方法:参加课程,听取教师讲解和案例 分析
高级微观经济学概述
章节副标题
微观经济学的定义和重要性
定义:微观经济 学是研究个体经 济单位(如家庭、 企业)的行为和 决策的学科。
重要性:微观经 济学可以帮助我 们理解市场机制 如何运作,以及 政府政策如何影 响经济。
应用:微观经济 学在商业决策、 政策制定、投资 分析等方面具有 广泛的应用价值。
交流与合作:与同学、老师、业 界专家等进行交流,共同探讨高 级微观经济学在实际经济问题中 的应用
高级微观经济学讲义(南京财大 桑乃泉)Jehle and Reny高级微观经济理论

1
e
0
x1
11
最后证明 u (x)是连续函数
效用函数在开区间上的原象 1 u ((a, b))
{x R a u(x) b}
n + n + n +
(定义)
{x R ae u(x)e be} (单调性) {x R ae x be} (传递性)
(ae) (be) 是开集
6
然后证明A、B为闭区间
任取t A, 若t >t, 那么t e te, 由 贩的严格单调性,t e te x, 因此,t A,从而A是一个闭区间 同理B也是一个闭区间,即 A= t ,+ , B 0, t
7
再证明A与B的交集非空
任取t 0, 由 · 的完备性, te 范x 或者te x, 所以t A, 或者t B 则 A B= 0, , + 从而 t t, A B非空
• 设 x * 0 在 (p0 ,y0 ) 0 下消费者的最优 选择。如果有
u (x) 是R 上的二次连续可微函数 u(x) / xi 0 i 1, 2,..., n
u (x) 加边海赛矩阵的秩不 等于0。
那么 x* (p0 , y 0 ) 在 (p0 ,y 0 ) 可微。
47
n
B 是凸集 x tx (1 t )x B
t 1 2
· :严格凸 u (x) 是严格拟凹函数
u(x ) min[u(x ), u(x )] u(x1 )
t 1 2
——与假设矛盾假设不成立解是唯一的
36
极值的唯一性:举例
非凸偏好
x2
x1 x2
张海洋高级微观经济学讲义

张海洋高级微观经济学讲义张海洋高级微观经济学讲义第一章:市场与竞争1.1 市场的定义和特征市场是指买方和卖方之间进行交易的地点和方式。
市场的特征包括:- 价格机制:市场上的价格是由供求关系决定的,价格上涨会促进供应量增加,价格下跌则会导致供应量减少。
- 自由进出:市场上任何人都可以自由进出,没有任何限制。
- 竞争:市场上存在多个卖方和买方,他们之间进行竞争。
1.2 市场失灵的原因市场失灵是指市场不能有效地分配资源。
常见的原因包括:- 外部性:当某个经济活动对于第三方产生影响时,就会出现外部性。
外部性可能是正面的(如教育)也可能是负面的(如污染)。
外部性会导致市场无法有效地分配资源。
- 公共物品:公共物品指不排除任何人使用、使用一个人不减少其他人使用量、使用者不需要付费等特征的物品。
公共物品容易出现免费骑车问题(free-rider problem),即有些人不付费却享受了公共物品的好处,导致市场无法提供足够的公共物品。
- 垄断:垄断是指市场上只有一个卖方或买方,他们可以控制价格和数量。
垄断会导致市场无法有效地分配资源。
第二章:消费者理论2.1 边际效用边际效用是指增加一单位消费量所带来的额外效用。
边际效用递减是指随着消费量增加,额外效用逐渐减少。
2.2 消费者最大化效用的条件消费者最大化效用的条件包括:- 边际效用相等原则:当两种商品的边际效用相等时,消费者达到了最优选择。
- 预算约束:消费者所能购买的商品数量受到收入和商品价格的限制。
- 无差异曲线:无差异曲线表示不同组合下产生相同总体效用的点。
在预算约束下,最大化效用意味着在无差异曲线上选择预算约束线上的点。
2.3 替代品和互补品替代品是指能够满足同样需求的不同商品。
互补品是指需要同时使用才能满足需求的商品。
第三章:生产者理论3.1 生产函数生产函数是指将输入转化为输出的关系。
生产函数可以表示为:Q = f(K,L)其中,Q表示输出量,K表示资本量,L表示劳动力。
高级微观经济学讲义

Chapter 1
36
消费者偏好和无差异曲线
无差异曲线
无差异曲线代表了能给一个人相同程度满足 的市场篮子的所有商品组合。
无差异曲线包含了全体给予某消费者同等满 意程度的消费组合。
Chapter 1
37
消费者偏好和无差异曲线
衣服(单位/周)
50
B
40
H
比起蓝色方框中的任一市场篮子, 消费者偏好于市场篮子A; 而比起市场篮子A,消费者偏好于 粉红色方框中的任一市场篮子。 (“多比少好”的第三个假定)
(PF = 1/2)
(PF = 2)
食物
40
80
120
Chapter 1
160 (单位/周)
28
预算约束
预算约束
• 两件商品和劳务
PX X PYY I , X 0,Y 0
• N件商品和劳务
P1 X1 P2 X 2 Pn X n I , X i 0, i 1,2, n
因而完整地描绘了某一消费者对于各种消费组合的偏好 态度。越是处于右上方的无差异曲线,所代表的满意程 度越高,越是处于左下方的无差异曲线,所代表的满意 程度越低。这些无差异曲线的全体叫做消费者的偏好映 照。
• 消费空间中,任意两条无差异曲线绝不会相交,也不平 行。
Chapter 1
44
消费者偏好和无差异曲线
边际替代率
消费者选择
消费者选择的运用
Chapter 1
21
预算约束
预算约束
• 购买力(有效需求)的大小构成了人们对于消费 品选择和购买的约束,而购买力(有效需求)则 取决于消费者的收入水平和物价水平。
Chapter 1
华中科技大学费剑平 高级微观经济学课程讲义 Lecture05
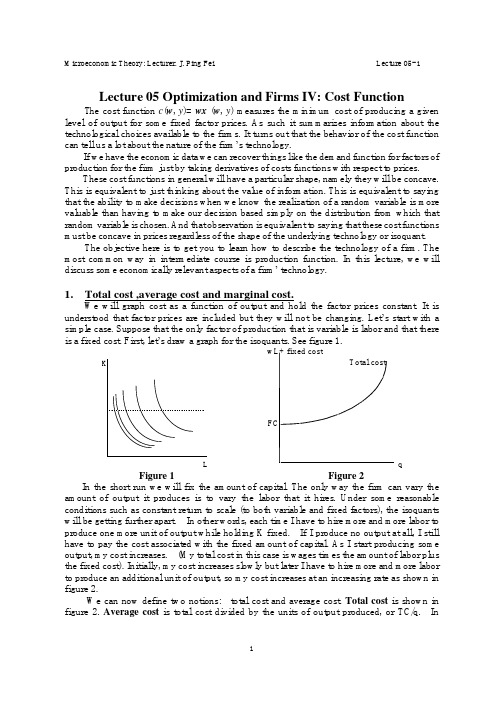
Microeconomic Theory: Lecturer. J. Ping FeiLecture 05- 1Lecture 05 Optimization and Firms IV: Cost FunctionThe cost function c(w, y)= wx (w, y) measures the minimum cost of producing a givenlevel of output for some fixed factor prices. As such it summarizes information about the technological choices available to the firms. It turns out that the behavior of the cost function can tell us a lot about the nature of the firm’s technology.If we have the economic data we can recover things like the demand function for factors of production for the firm just by taking derivatives of costs functions with respect to prices.These cost functions in general will have a particular shape, namely they will be concave. This is equivalent to just thinking about the value of information. This is equivalent to saying that the ability to make decisions when we know the realization of a random variable is more valuable than having to make our decision based simply on the distribution from which that random variable is chosen. And that observation is equivalent to saying that these cost functions must be concave in prices regardless of the shape of the underlying technology or isoquant.The objective here is to get you to learn how to describe the technology of a firm. The most common way in intermediate course is production function. In this lecture, we will discuss some economically relevant aspects of a firm’ technology.1. Total cost ,average cost and marginal cost.We will graph cost as a function of output and hold the factor prices constant. It is understood that factor prices are included but they will not be changing. Let’s start with asimple case. Suppose that the only factor of production that is variable is labor and that there is a fixed cost. First, let’s draw a graph for the isoquants. See figure 1.wL+ fixed costKTotal costFCLqFigure 1Figure 2In the short run we will fix the amount of capital. The only way the firm can vary theamount of output it produces is to vary the labor that it hires. Under some reasonableconditions such as constant return to scale (to both variable and fixed factors), the isoquantswill be getting further apart. In other words, each time I have to hire more and more labor toproduce one more unit of output while holding K fixed. If I produce no output at all, I stillhave to pay the cost associated with the fixed amount of capital. As I start producing someoutput, my cost increases. (My total cost in this case is wages times the amount of labor plusthe fixed cost). Initially, my cost increases slowly but later I have to hire more and more laborto produce an additional unit of output, so my cost increases at an increasing rate as shown infigure 2.We can now define two notions: total cost and average cost. Total cost is shown infigure 2. Average cost is total cost divided by the units of output produced, or TC/q. In1Microeconomic Theory: Lecturer. J. Ping FeiLecture 05- 2terms of figure 2, average cost is the slope of the ray from the origin to the total cost curve.This would be the slope of the diagonal lines in Figure 2. When output is close to zero,average cost is really big (the slope is really steep). If my total cost is $10,000 and I produceone unit of output, my average cost is $10,000. If I only produce 1/2 unit of output, my average cost is $20,000, assuming the variable cost of ½ unit and 1 unit are the same. Asoutput increases, the slope decreases until it reaches a minimum. Beyond that minimum,average cost starts to rise again. So, my average cost curve might be expected to have somekind of a U-shape (see figure 3).Marginal cost. Marginal cost is the cost of producing one more unit of output. Marginalcost is the derivative of total cost with respect to output (q). As output increases, the slope ofmarginal cost gets steeper. Since capital is fixed (say capital is the amount of machines Ihave), the only way I can increase my output is by hiring more unit of labor. However, themore people I hire to work with the same number of machines, the less additional output Iwill be getting from each additional unit of labor. Eventually, I will run into some capacitylimitation and my output will be very difficult to increase.MCACTotal cost for the small factoryTotal cost for the large factoryqFigure 3Figure 4The marginal cost curve goes through the minimum of the average cost curve (see figure 3)because the minimum average cost is where the average cost is tangent to total cost (seefigure 2), and the slope of the tangent to total cost is marginal cost.For the intuition, think about bowling scores. You know that if you score less than youraverage, then you lower your average and if you score more than your average, then you raiseyou average. If your average stays the same, it must be because your score on the last gamewas the same as your average.Long run total cost and short run total cost. In the long run I can choose the amount ofthe fixed input, capital in this case. For example, I can choose between a small factory and alarge one. In terms of the graph, the total cost curve of a small factory will have a lower levelof fixed cost than the large factory. However, the total cost curve of the small factory willrise faster than the total cost curve of the larger factory (see figure 4).Which factory would I rather have? It would depend on how much I want to produce. IfI want to produce any amount up to where the short run total cost curves intersect, I wouldrather have the smaller factory because it produces it more cheaply. On the other hand, if Iwant to produce a large amount of output, then I want to have the larger factory because itproduces that level of output at a lower cost. If these two factories are my only alternatives,in the long run my total cost curve would look like the thick line in figure 4.If we have more alternatives in terms of size of factories (each alternative has a differenttotal cost curve), in the long run the cost of any particular level of output is going to be thecost associated with the cheapest means of producing that level of output. The long run totalcost curve will be the lower bound of all the short run total cost curves (see figure 5).2Microeconomic Theory: Lecturer. J. Ping FeiLecture 05- 3SRTCSRTC (1) SRTC LRTCAC (1)AC (3) AC (2)LRACqq11Figure 5Figure 6The LRTC curve is just the lower ‘envelope’ of all the SRTC curves. The LRTC curvedoes not have to start at the origin if there are fixed costs. For any particular set of fixedfactors, short run total cost is the minimum total cost over all of the factors that are variable.Long run total cost for a given level of output is the minimum of short run total cost overfixed factors of production given the level of output. And this is equal to the minimum (overfixed factors) of the minimum (over variable factors) of total cost given q.The question is in what order we are doing the minimization. We turned this into atwo-stage problem. My costs depend not only on the level of output but also on how much ofthe variable factors I hire and how much of the fixed factors I hire. We are defining short runcost as what you get when you minimize over the variable factors. And we are defining longrun cost as what you get when you minimize the short run cost over the fixed factor. What weend up with is a long run total cost obtained by minimizing costs over both fixed and variablefactors. The order of the minimization does not matter.Long run average cost and short run average cost. Figure 6 shows the average costcurves corresponding to the total cost curves in figure 5.short-run total cost=STC=wv xv (w, y, xf ) + wf x fshort-run average cost=SAC=c(w, y, xf ) / yshort-run average variable cost=SAVC=wv xv (w, y, xf ) / yshort-run average fixed cost=SAFC=wf x f / yshort-run marginal cost=SMC=∂c(w, y, x f ) / ∂ylong-run average cost=LAC=c(w, y) / ∂ylong-run marginal cost=LMC=∂c(w, y) / ∂yTo derive the long run and short run average cost curves, suppose we choose a certain level of output, say q1. At output level q1, long run total cost was obtained by choosing the minimum of all ways of producing q1. Output level q1 was produced at lowest cost by factory 1. At q1, the average total costs are the same in the short run and the long run. If factory 1 produces at any level of output different from q1, short run total costs are higher than long run total costs, and hence short run average costs are higher than long run average costs.We can repeat this analysis with factories 2 and 3. So the long run average cost curve is the envelope of the short run average cost curves, in the same way that my long run cost curve is the envelope of the short run total cost curves. Also, long run and short run average costs will be the same for the output level for which the particular factory is the least costly at producing the given level of output.3Microeconomic Theory: Lecturer. J. Ping FeiLecture 05- 4Note that the place where the SRAC curves and the LRAC curve are tangent is notnecessarily the minimum of each SRAC curve. When the LRAC curve is declining thetangency point occurs before the minimum of the SRAC curve and where the LRAC curve isincreasing, the tangency point occurs beyond the minimum of the SRAC curve. The onlypoint where the tangency point occurs at the minimum of a SRAC curve is at the minimum ofthe LRAC curve.This may sound confusing because we are saying that to produce a level of output q1, the best plant to use is plant 1. However, q1 is not the best level of output for that particular plant. Plant 1 could produce a higher level of output at a lower average cost. But if we want toproduce at that higher level of output, plant 1 would not be the best plant to use.We are not interested in the level of output that a plant produces most cheaply for itself.Instead, we are interested in how well that plant produces a particular level of output, relativeto other plants.If I want to produce 100 units of output and plant 1 produces the 100 units of output atthe lowest cost, I do not care if plant 1 can produce 110 units at a lower average cost. What Icare about is which plant produces 100 units in the cheapest way.A baseball analogy: I am interested in putting the best second baseman at second base.The fact that he may be a better third baseman does not interest me if I have a guy who playsthird base still better than he. The fact that you do third base best does not interest me if youhappen to be the best second base player I have and if I have a guy who can play third basebetter than you can.To summarize, what is important to you is not running a plant at its minimum averagecost. What is important to you is deciding what level of output you want to produce anddeciding how to produce that level of output in the cheapest way.How do we decide what level of output I want to produce? Suppose I can sell myoutput at price p per unit of output. If I sell q units, my revenue is pq. My profit is my revenueminus my cost. Graphically, my profit is the vertical difference between the revenue line andthe total cost curve (it does not matter if it is short run total cost or long run total cost) (seefigure 7).$TC Revenue (pq)LRMC LRACNegative profitsPPositive profitsqq*q*Figure 7Figure 8If I want to maximize my profit, I have to choose the point where the curves are thefurthest apart, that is, where the slopes are the same. Since the slope of the revenue line is theprice of output, and the slope of the total cost curve is marginal cost, I maximize my profitwhere price equals marginal cost (where output = q* in figure 7). If I produce less than that,price is greater than marginal cost and I can make more money by producing more. If Iproduce at a point where marginal cost is greater than price, then I can make more money byproducing less.4Microeconomic Theory: Lecturer. J. Ping FeiLecture 05- 5In figure 8, I maximize my profit if I produce at the level of output where the LRMC is equal to the price (at q*).So, I want to produce a level of output q*, and I want to produce it in the cheapest way. The factory associated with the SRAC curve in figure 8 is the factory that produces q* in the cheapest way. In this case, q* is at a point beyond the minimum for that factory.2.Examples:1). The short-run Cobb-Douglas cost functions. min w1x1 + w2 x2 s.t. x2 = k and y = x1a x12−a1−a⇒ SAC = w1( y / k) a + w2k / y1−aSMC=w1( y / k) a / a Proposition 1: Constant returns to scale. If the production function exhibits constant returns to scale, the cost function may be written as c(w, y)= y c(w,1).Proof: let x* be a cheapest way to produce one unit of output at prices w. Notice that yx* is feasible to produce y since the technology is constant returns to scale. Suppose it does not minimize cost; instead let x′ be the cost-minimizing bundle to produce y at w so wx′/y<wyx*/y. Then x′/y can produce 1 since the technology is constant returns to scale.Proposition 2: Marginal costs equal average costs at the point of minimum average costs.Proof: Let y* denote the point of minimum average cost, then to the left of y* averagecosts are declining so that for y≤y* d (c( y) / y) / dy ≤ 0 which implies c′( y) ≤ c( y) / y ∀y ≤ y * . A similar analysis shows that c′( y) ≥ c( y) / y ∀y ≥ y * .Proposition 3:limy→0cv( y) y= cv′ (0)2). CD cost curves1c( y) = Ky a+b1− a −bAC( y) = Ky a+bMC( y) = c′( y) =K1−a −by a+ba+bProposition 4: The long- and short run (average) cost curves are tangent.3. Factor prices and cost functionsProperties of the cost function (1) Non-decreasing in prices. If w′≥w, then c(w′, y) ≥c(w, y). (2) Homogeneous of degree 1 in w. c(tw, y)= t c(w, y) for t>0. (3) Concave in w. c(tw + (1− t)w′, y) ≥ tc(w, y) + (1− t)c(w′, y) ∀0 ≤ t ≤ 1(4) Continuous in w. c(w, y) is continuous as a function of w for w ? 0. (5) Shephard’s lemma. (the derivative property.) Let xi(w, y)be the firm’s conditional factor demand for input i. Then if the cost function is differentiable at (w, y), and wi>0 for i=1, …, n,5Microeconomic Theory: Lecturer. J. Ping FeiLecture 05- 6then xi (w, y) = ∂c(w, y) / ∂wi i = 1,L, n . Proof: Construct g(w)= c(w, y)−wx*, since is thecheapest way to produce y, ∂g(w)/∂wi=0. We can also prove it by the definition of c(w, y)= wx(w, y) or directly by the envelopetheorem. The concavity of the cost function is also a nice geometrical argument.4. The envelope theorem for constrained optimizationdM (a) = ∂L(x, a)da∂ax= x(a )=∂g ∂ax=x(a)−λ∂h ∂aApplications: Shephard’s lemma⇒∂c(w, ∂wiy)=xixi = xi ( w, y )=xi (w,y),marginal cost L = w1x1 + w2 x2 − λ[ f (x1, x2 ) − y] ∂c(w1, w2 , y) / ∂y = λ5. Comparative statics using the cost function1) The cost function is nondecreasing in factor prices⇔ Factor demand x(w,y) is nonnegative. 2) The cost function is homogeneous of degree 1 in w ⇔ x(w,y) is homogeneous of degree 0. 3) The cost function is concave in w has the following implications.a) the cross-price effects are symmetric. b) the own-price effects are nonpositive. c) dwdx≤06。
高级微观经济学博弈论讲义(复旦大学CCES Yongqin Wang)

Player 1 s12 s13
What is game theory?
We focus on games where: There are at least two rational players Each player has more than one choices The outcome depends on the strategies chosen by all players; there is strategic interaction Example: Six people go to a restaurant. Each person pays his/her own meal – a simple decision problem Before the meal, every person agrees to split the bill evenly among them – a game
At the separate workplaces, Chris and Pat must choose to
attend either an opera or a prize fight in the evening. Both Chris and Pat know the following:
Dec, 2006, Fudan University
Game Theory--Lecture 1
11
Definition: normal-form or strategicform representation
The normal-form (or strategic-form)
representation of a game G specifies:
高级宏微观经济学讲义(吴120313)

三.最优化方法
(一) 静态最优化问题 1.无约束的静态最优化问题
MinV f ( x )
x
最优化问题的解:
V x f ( x) 0 2 V f ( x) 0 x 2
2.有约束的静态最优化问题 (1)等式约束 1)一个内生变量
问题:
MinV F ( x )
f ( x1 ) (1 ) f ( x2 ) f [ x1 (1 ) x2 ]
则, f 为凸函数.
严格凸函数:
f ( x1 ) (1 ) f ( x2 ) f [ x1 (1 ) x2 ]
凹函数:
f ( x1 ) (1 ) f ( x2 ) f [ x1 (1 ) x2 ]
L x1x2 2x1 (60 4x1 2x2 )
充分条件: 指约束条件对x1,x2…求一阶导!
构造如下的Hesse加边行列式:
0 h1 H h2 hn
h1 F11 F21 Fn 1
h2 F12 F22 Fn 2
Matlab软件实现 某商品价格变化规律为:
P 5.6 1.4 P
求解程序:
dsolve(‘Dp=5.6-1.4*p’, ‘p(0)=1’) 默认的自变量t。可以得到解析式
(4) 一阶线性变系数微分方程:
y(t ) a(t ) y(t ) x(t ) 0
解析解:
y(t ) e 0
t
a ( ) d
e0
t
a ( ) d
0 a ( ) d x(t )dt C e
t
例:
y(t ) t 2 t y(t ) 1 y(0) 10
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
,,
使得 0且∑
1。
定理 1.17 消费者需求上的加总
设 , 是消费者的马歇尔需求;对所有的 , 1, … , , 、 和 如 上定义。则收入份额、价格和需求的收入弹性之间有如下关系:
1. 恩格尔加总:∑
1
2. 古诺加总:∑
,
1, … ,
说明:给定 0,则对每个 ≫ ,
⋅,
。
证明:根据预算平衡性,
,…, ,…, ; ≡
给定假设 1.2,消费者的需求函数在价格和收入上零阶齐次;并且, 对所有的 , ,满足预算平衡性。
首先证明零阶齐次性。
证明:取 , ,取 0。设在 , 和 , 下消费者的需求分别
为
,和
, 。我们要证明它们相等。 是价格和收
入为 , 时的解,因此,
因此,对所有的 0,
⋅
。
⋅
。
就是说,在价格和收入为 , 时,消费者买得起 。因此,
证明:根据谢菲尔德引理,对所有的 1, … ,
∂ ∂
,
∂∂ ,
∂
∂
∂ ∂∂
,
因此,
∂ ∂
,
⋯
∂ ∂∂
,
σ, ≡
⋮
⋱
⋮
≡
,
∂ ∂∂
,
⋯
∂ ∂∂
,
因为支出函数是 的凹函数,因此,其海赛矩阵 以,矩阵σ , 负半定。
, 负半定,所
证毕
定理 1.16 Slutsky 矩阵为对称矩阵和负半定矩阵 设 , 是消费者的马歇尔需求。定义第 个 Slutsky 项为
, ,令 ∗ ≡
, 。根据
,, ≡ ,
根据定理,
,,
,∗
因此,
,, ≡ ,,,
带入 ∗,得到
,∗≡
, ,∗
这个式子对所有的 ≫ 都成立。等号两边对 求导, 到
1, … , ,得
∂ ∂
,∗
∂ ∂
, ,∗
∂ ∂
,
,∗
∂ ∂
,∗
应用谢菲尔德引理,得到
∂ ∂
,∗
∂ ∂
, ,∗
∂ ∂
, ,∗
,,
∂ ∂
, ,∗
4
∂, ∂
,
∂, ∂
,
并把价格和收入反应的整个 Slutsky 矩阵写为
, ∂,
∂
∂, ∂
,
∂, ∂
⋮
,
∂, ∂
⋯
∂, ∂
⋱
⋯
∂ ∂
,
,
∂, ∂
⋮
。
,
∂, ∂
则 , 是对称矩阵,也是负半定矩阵。
定义 1.6 需求弹性和收入份额
设 , 是消费者对商品 的马歇尔需求。设
≡∂
, ∂
,
≡∂ ∂ ,
,
设
≡
,
在只消费两种商品的情况中,至少一种商品必须为正常商品。
证明:根据预算平衡性,
,…, ,…, ; ≡
恒等号两边对 求导,得到
,…, ,…, ;
,…, ,…, ; 0
整理得到
,…, ,…, ;
,…, ,…, ;
现在,凑弹性表达式:等号两边同乘以 ,得到
得到
,…, ,…, ;
,…, ,…, ;
6
因此,支出函数的自二阶导数非正,即对所有的 1, … , ,
∂, ∂
0
根据谢菲尔德引理,
∂, ∂
,
于是,
,
∂, ∂
0
定理 1.13 需求法则
正常商品自身价格下降,将导致其需求量上升。如果一种商品自身价 格的下降,导致其需求量下降,则这种商品肯定是低档商品。
定理 1.14 替代项对称
设 , 是消费者的希克斯需求,设支出函数 二阶连续可微,则对 所有的 , 1, … , ,
∂ ∂
, ,∗
,
整理得到:
2
∂ ∂
,
∂ ∂
,∗
,
∂ ∂
,
说明:
证毕
∂, ∂ ⋮
∂, ∂
⋯
∂ ∂
,
⋱
⋮
⋯
∂ ∂
,
∂, ∂
∂, ∂
,
∂, ∂
⋮
,
∂, ∂
⋯
∂, ∂
⋱
⋯
∂ ∂
,
,
∂, ∂
⋮
,
∂, ∂
定理 1.12 自替代项为负 设 , 是对商品 1, … , 的希克斯需求。则
∂, ∂
0,
1, … ,
证明:定理 A2.5 说,当凹函数二阶连续可微时,自二阶导数非正。 而定理 1.6 说,支出函数 , 在 上是凹函数。
算线上,就是说,
⋅,
证毕
定理 1.11 Slutsky 方程
设 , 是消费者的马歇尔需求。设 ∗是在价格为 和收入为 时,消 费者实现的效用水平。则对所有的 , 1, … , ,
∂, ∂
全部效应
∂ ,∗ ∂
替代效应
证明:给定 , ,则间接效用函数为 定理,对所有的 1, … , ,
,
∂, ∂
收入效应
恒等号两边对 求导,得到
,…, ,…, ; 1
5
整理得到 ,…, ,…, ;
1 得到
,…, ,…, ;
,…, ,…, ;
1
证毕
就是说,以支出份额为权重,各商品的收入弹性的平均值为 1。
假设所有商品都是低档品,即随着收入上升,需求量下降的商品。这
些商品的需求的收入弹性小于零。∑
0。矛盾。因此,不可
能所有商品都是低档品。
。 另一方面, 是价格和收入为 , 时的解,因此,
因此,
⋅
。
⋅
。
就是说,在价格和收入为 , 时,消费者买得起 。因此,
。
我们于是得到
。
假设 1.2 指出效用函数严格拟凹,因此,
1
就是说,
,
,。
证毕
现在证明预算平衡性。
证明:任取价格和收入 , ,设最优解为 ∗
, 。根据假设 1.2,
效用函数严格递增。消费者因此会耗尽全部收入,最优解必然位于预
3
∂, ∂
∂, ∂
证明:交叉导数的求导顺序不重要,因此,
∂∂ ,
∂
∂
∂∂ ,
∂
∂
根据谢菲尔德引理,
∂, ∂
,
∂, ∂
,
因此,
∂ ∂
,
∂ ∂
,
定理 1.15 替代矩阵负半定 设 , 是消费者的希克斯需求,设
证毕
∂, ∂
⋯
∂ ∂
,
σ, ≡
⋮
⋱
⋮
,
∂, ∂
⋯
∂ ∂
,
称为替代矩阵,包含所有的希克斯替代项。则矩阵σ , 负半定。
第五次课
第四次课需要掌握的内容
1. 知道最大值定理
2. 能运用包络定理 3. 间接效用函数,间接效用函数的性质 4. 支出函数,支出函数的性质
5. 效用最大化问题与支出最小化问题之间的对偶关系 需求函数 , 的特征
主要探讨课本第 62 页 Figure 1.21 中的结论。
定理 1.10 零阶齐次性和预算平衡性
