2005滑铁卢竞赛试题答案
2005PGG真题答案

2005I. DiktatVerkehrsmittelDie Menschen interessieren sich für die neueren Verkehrsmittel,besonders für das Auto und das Flugzeug.Wie steht es aber mit der Eisenbahn? Sie ist nicht so frei verwendbar wie der Kraftwagen.Sie kann sich nicht von der Erde erheben wie das Flugzeug.Aber sie kann lange ZügeBilden und große Lasten bewegen.Und sie kann sich für ihren Betrieb in viel größerem Maße als andere Verkehrsmittel der Automation bedienen,wobei die Technik der Elektronik eine wichtige Rolle spielt.Der elektrische Betrieb eröffnet der Eisenbahn die Möglichkeit,sich in Zukunft sogar der Kernenergie zu bedienen.Nach dem heutigen Niveau der Technik arbeitet kein Verkehrsmittel so wirtschaftlich wie die Eisenbahn.In Zukunft wird die Eisenbahn mit Sicherheit weitere technische Fortschritte machen und der Menschheit noch besser dienen.II Hörverstehen (Hörtexte)Teil 1 Alltagssituationen1. A: Entschuldigung,wann fährt der Zug ab?B: In 15 Minuten.A: Wie spät ist es jetzt?B: Es ist halb elf.Frage: Wann fährt der Zug ab?2. (T = Monika, W= Wolfgang)W:Also,Monika,endlich hast du ein Zimmer im Studentenheim.Sechs Monate hat es gedauert.T: Ja,ich bin froh.Wolfgang,kannst du mir beim Einrichten helfen?W: Sicher,gern! Wann wird das sein?T: Am besten gleich,Dann kann ich morgen einziehen.W:O.K. Dann fahren wir jetzt hin.Frage: Wobei hilft Wolfgang Monika?3. A: Ein Sauwetter,findest du nicht?B: Typisch,ist ja Wochenende.Am Montag wird es bestimmt wieder schöner.Frage: Welche Aussage ist richtig?4. (A= Student, B= Studentin)A: Wohnst du noch bei deinen Eltern?B: Nein,ich musste ausziehen,weil ich ja jetzt in Münster studiere.A: Hast du eine eigene Wohnung?B: Nein,ich wohne mit zwei anderen Studentinnen zusammen.Frage: Welche Aussage stimmt?5. (A= Peter, B= Kathrin)A: Kathrin,kommst du heute mit ins Konzert?B: Tut mir leid,Peter,heute kann ich nicht.A: Schade.Warum nicht?B: Ich muss mit meiner kleinen Schwester in die Disko gehen,obwohl ich dazu überhaupt keineLust habe. Aber sie darf abends nicht allein weg,da muss ich halten manchmal mit.Frage: Warum kommt Kathrin nicht mit ins Konzert?6. (A= Journalistin, B= ein Passant)A: Entschuldigung,haben Sie einen Traumberuf?B: Wie bitte?A: Einen Traumberuf.B: Den habe ich vielleicht schon.Weiß ich nicht.A: Was sind Sie denn von Beruf?B: Ich bin Geschäftsführer des philharmonischen Orchesters in Dortmund.A: Das ist,glaube ich,wirklich ein Traumberuf.Danke schön.B: Bitte schön.Frage: Welche Aussage ist richtig?Teil 2Text A Freizeit und Urlaub in DeutschlandViele Unternehmen geben ihren Mitarbeitern sechs Wochen Urlaub.Außerdem zahlen sie oft noch ein Urlaubsgeld.Die Schulkinder haben im Sommer auch sechs Wochen Ferien.Nach Umfragen gibt jede Familie in Deutschland etwa 20% ihres Einkommens für den Urlaub oder die Freizeit aus.Im Jahr 1999 haben etwa 70% der Bundesbürger eine Urlaubsreise von fünf Tagen unternommen.Sie verbringen zu 50% ihre Freizeit und ihren Urlaub im Inland.Sie fahren gerne an die See nach Norddeutschland oder in die Berge nach Süddeutschland.Manche fahren ins Ausland. Sie wollen ein Stück von der Welt sehen.Unter den Reisezielen liegen die Nachbarstaaten wie Österreich,Frankreich,die Schweiz und Italien,sowie Spanien und die USA an der Spitze.Die Bundesbürger wollen sich während ihres Urlaubs nicht nur erholen,sondern auch bewegen.Heute ziehen viele Menschen einen Urlaub mit viel Bewegung vor. Sie wandern,schwimmen,fahren Rad,laufen Ski oder klettern auf Berge.Text B Wie sehen Sie die Zukunft des Buches?Bücher werden immer mehr gelesen,trotz der modernen Medien.Viele junge Leute surfen im Internet,viele Kinder wachsen ohne Bücher auf,sie beschäftigen sich eher mit Computerspielen,jeder Erwachene hat mittlerweile ein verkabeltes Fernsehgerät und kann zwischen 20 und 50 Fernsehprogrammen empfangen,und dennoch wird immer wieder gelesen werden.Deswegen ist es wichtig,für Bücher Werbung zu machen – ohne Werbung läuft nicht viel.Da muss man schon inverstieren,Akzente setzen,damit man heutzutage auffällt.Früher wurde für Bücher sehr wenig Werbung gemacht,da hatte man eine Schaufensterdekoration oder ein paar Zeitungsanzeigen,heutzutage geht man andere Wege,auch in unserem Verlag.Wir produzieren Hörfunk-Werbetexte und Kino-Werbetexte und werden jetzt bald im Fernsehen mit Werbung zu sehen sein.Also,wir gehen auch mit der Zeit,weil wir wissen,wo die Leute ihre Anregungen bekommen.Text C Interview mit einer Japanerin(A= ein Deutscher, B= eine Japanerin)A: Du hast anfangs bei einer deutschen Familie gewohnt.Konntest du dich dort schnell …einleben“?B: Die Familie war wirklich sehr nett zu mir.Aber ich musste mich immer sehr deutlich und wörtlich äußern.Das war ichnicht gewohnt.In Japan brauche ich nicht immer alles so direkt und klar zu sagen.Sie versuchen mich so zu verstehen.A: Das verstehe ich,glaube ich,nicht ganz......B: Tja zum Beispiel,wenn wir was angeboten wird,was ich auch gerne essen würde,dann sage ich in Japan trotzdem zuerst nei.Ich werden dann noch mehrmals danach gefragt.Und dann allmählich sage ich: …Achja,vielleicht doch,bitte.“In Deutschland dagegen muss ich immer kategorisch mit …ja“oder …nein“ antworten.A: Wie wirkt denn diese Direktheit auf dich?B: Zuerst habe ich mich sehr gefreut,dass ich endlich offen meine Meinung äußern durfte.In Japan muss ich oft das Wort runterschlucken.Ich kann selten meine Meinung offen sagen.Das hat etwas mit Rücksicht zu tun,die nicht selten übertrieben ist.Man soll deshalb einfach zurückhaltend bleiben.Hier darf ich nun alles offen sagen und es hat mir am Anfang auch sehr gut gefallen.Doch nach und nach ging es mir auf die Nerven.A: Warum denn?B: Das liegt wahrscheinlich an der Sprache oder an der Logik. Etwas deutlich und klipp und klar zu sagen,ist immer einfach und auch verständlicher.Aber des verletzt den anderen und der andere verletzt mich auch.Und es muss ja nicht sein.Text D Auf der Wohnungssuche(Telefonanruf,Haller =Mann, Neumann= Frau)A: Haller.B: Neumann,guten Tag.Ich habe heute Morgen Ihre Anzeige in der Zeitung gelesen.Sie suchen einen Nachmieter für eineDreizimmerwohnung in Ottensen,nicht wahr?A: Ja,das stimmt.Es ist eine 70-Quadratmeter-Wohnung mit Bad,Küche und einem kleinen Balkon.Die Miete beträgt 700 Euro.B: Kalt oder warm?A: Kalt.B: Und die Wohnung ist noch zu haben?A: Ja,Sie sind heute der dritte Anrufer.Eine Dame wird heute Nachmittag vorbeikommen.Zugesagt habe ich die Wohnung noch niemandem.Das kann ich ja auch nicht,ich bin ja nur der Mieter.B: Können Sie mir ungefähr sagen,wie hoch die Nebenkosten sind?A: Ja,natürlich,das kann ich Ihnen ziemlich genau sagen: 70 Euro für Gas und Strom,monatlich.Es hängt natürlich von Ihrem Verbrauch ab.Ich weiß nicht,mit wie vielen Personen Sie einziehen,also,ich bin allein stehend.Dann kommt da noch ein Betrag von ungefähr,also das ändert sich oft,also ungefähr 180 Euro vierteljährlich hinzu,für Wasser,Müllabfuhr,und ich weiß nicht was noch alles.B: Und das Haus ist in Ordnung? Sie sagten,es ist ein Altbau......?A: Sehr gut.Selbst die Fassade wurde vor 2 Jahren gestrichen.Naja,und Sie sind ja noch jung,das hört man ja an der Stimme,die Wohnung ist nämlich in der 4.Etage.B: Gibt es da einen Aufzug?A: Nein,leider nicht.B: Wie schade,dann passt sie mir doch nicht so.Ich habe nämlich zwei kleine Kinder,und mit ihnen und demKinderwagen die Treppen fünfmal am Tag rauf und runter,ich weiß nicht,ob das so ideal wäre.A: Ja,das müssen Sie wissen.Die Kinder werden ja auch mal größer.Aber ich will Sie da um Gottes Willen nicht überreden.Sie wissen ja wahrscheinlich selber,dass es nicht so ganz einfach ist,in Hamburg eine Wohnung zu finden.B: Ja,sicher,ich überlege es mir mal.Vielen Dank!II. Hörverstehen (Lösungen)III: LesenverstehenV. Wort,Satz,TextB.55.wie 56.Mein(e)s 57.dass 58. getroffen 59.genannt 60.sich 61.abhängig 62.von 63.würde 64.einem 65.obwohl 66.geschrieben 67.daran 68.leicht 69.trotzdem 70.Auf 71.billiger/günstiger 72.scheint 73.statt/als 74.Schluss/FeierabendC.75.jeder 76.von 77.zu 78.Stuhl 79.was 80.sich 81.Grund 82.sitzen 83.Kinder/Sie 84.tragen 85.entwickelt 86.Zu 87.der/aller/von 88.dass 89.wächst/(an)steigt 90.Internet 91.von 92.von 93.bezeichnete 94.nurD95.Es war im 18. Jahrhundert völlig üblich,dass die Adligen das Französische verwendete.96.Damit Nachrichten möglichst schnell verbreitet werden(können),haben die Menschen verschiedene Informationssysteme eingerichtet/Um Nachrichten möglichst schnell zu verbreiten.97.Als/Nachdem sie aus dem Urlaub zurückgekehrt waren,erzählten sie allen Bekannten von ihrer abwechslungsreichen Reise.98.Dem seit acht Jahren fliegenden Piloten ist noch nie etwas Ähnliches passiert.E(Vorschläge)99. ......,diskutieren über Sort/machen Musik.100. ......,aber Kinder können durch Fernsehen auch viel lernen.101. ......,Deshalb mache ich jetzt auch einen Computerkurs.102. ......,sie haben es sich geliehen.。
2005滑铁卢竞赛试题

2. Finished solutions must be written in the appropriate location in the answer booklet. Rough work should be done separately. If you require extra pages for your finished solutions, foolscap will be supplied by your supervising teacher. Insert these pages into your answer booklet. Be sure to write your name on any inserted pages. 3. Marks are awarded for completeness, clarity, and style of presentation. A correct solution poorly presented will not earn full marks. NOTE: At the completion of the Contest, insert the information sheet inside the answer booklet.
y
C x B(2, 0) A(0, 1)
(c) In the diagram, triangles AOB and CDB are right-angled and M is the midpoint of AC . What are the coordinates of M ?
y
A 50 O
2.
M C 50 B 48 D x
NOTES: 1. Please read the instructions on the front cover of this booklet. 2. Write all answers in the answer booklet provided. 3. For questions marked “ ”, full marks will be given for a correct answer placed in the appropriate box in the answer booklet. If an incorrect answer is given, marks may be given for work shown. Students are strongly encouraged to show their work. 4. All calculations and answers should be expressed as exact numbers such as √ 4π , 2 + 7, etc., except where otherwise indicated. 1. (a) If the point (a, a) lies on the line 3x − y = 10, what is the value of a? (b) In the diagram, points A, B and C lie on a line such that BC = 2AB . What are the coordinates of C ?
滑铁卢竞赛数学题

滑铁卢竞赛数学题概述
滑铁卢竞赛数学题通常比较难,涉及的知识点广泛,包括代数、几何、数论、组合数学等多个领域。
以下是一些滑铁卢竞赛数学题的示例:
1. 有100个球,其中有一个与其他99个重量不同,但外观相同。
用一个天平,最少需要称多少次才能确定这个重量不同的球?
2. 一个正方形的面积为1,将其四边中点连接起来,形成另一个正方形。
如此重复,得到第五、第六个正方形,求第五个正方形的面积。
3. 一个圆被分成n个相等的扇形,其中一个是空心的,其他n-1个是实心的。
求空心扇形的圆心角是多少度?
4. 有100个人站成一排,从第1个人开始报数,每次报到奇数的人离开队伍。
经过若干轮后,只剩下一个人。
求这个人最初站在第几位?
5. 有5个不同质因数的最小正整数是多少?
以上仅是滑铁卢竞赛数学题的一些示例,实际上还有更多难题和技巧题。
如果想要深入了解滑铁卢竞赛数学题的解题技巧和策略,建议参考相关的竞赛书籍和资料,或者参加专业的数学竞赛培训课程。
2005年全国高中数学联赛试题(二)及参考答案

2005年全国高中数学联赛试题(二)及参考答案一、(本题满分50分) 如图,在△ABC 中,设AB>AC ,过A 作△ABC 的外接圆的切线l ,又以A 为圆心,AC 为半径作圆分别交线段AB 于D ;交直线l 于E 、F 。
证明:直线DE 、DF 分别通过△ABC 的内心与一个旁心。
(注:与三角形的一边及另两边的延长线均相切的圆称为三角形的旁切圆,旁切圆的圆心称为旁心。
) 证明:(1)先证DE 过△ABC 的内心。
如图,连DE 、DC ,作∠BAC 的平分线分别交DC 于G 、DE 于I ,连IC ,则由AD=AC , 得,AG ⊥DC ,ID=IC. 又D 、C 、E 在⊙A 上, ∴∠IAC=21∠DAC=∠IEC ,∴A 、I 、C 、E 四点共圆, ∴∠CIE=∠CAE=∠ABC ,而∠CIE=2∠ICD , ∴∠ICD=21∠ABC.∴∠AIC=∠IGC+∠ICG=90°+21∠ABC ,∴∠ACI=21∠ACB ,∴I 为△ABC 的内心。
(2)再证DF 过△ABC 的一个旁心.连FD 并延长交∠ABC 的外角平分线于I 1,连II 1、B I 1、B I ,由(1)知,I 为内心, ∴∠IBI 1=90°=∠EDI 1,∴D 、B 、l 1、I 四点共圆, ∵∠BI l 1 =∠BDI 1=90°-∠ADI 1=(21∠BAC+∠ADG )-∠ADI=21∠BAC+∠IDG ,∴A 、I 、I 1共线. I 1是△ABC 的BC 边外的旁心二、(本题满分50分)设正数a 、b 、c 、x 、y 、z 满足.;,c ay bx b cx az a bz cy =+=+=+求函数zz y y x x z y x f +++++=111),,(222的最小值. 解:由条件得,0)()()(=-+--++-+a bz cy a c ay bx c b cx az b ,即02222=--+c b a bcx ,bca cb x 2222-+=∴,同理,得.2,2222222ab c b a z ac b c a y -+=-+=a 、b 、c 、x 、y 、z 为正数,据以上三式知,222222222,,c b a b c a a c b >+>+>+,故以a 、b 、c 为边长,可构成一个锐角三角形ABC ,C z B y A x cos ,cos ,cos ===∴,问题转化为:在锐角△ABC 中,求函数A f (cos 、B cos 、C cos )=CCB B A A cos 1cos cos 1cos cos 1cos 222+++++的最小值.令,cot ,cot ,cot C w B v A u ===则,1,,,=++∈+wu vw uv R w v u且).)((1),)((1),)((1222w v w u w w v v u v w u v u u ++=+++=+++=+1)1()1(1111cos 1cos 2222222222+-+=+++=+++=+∴u u u u u u u u u u u u AA),11(2))((13232232w u v u u u w u v u u u u u u +++-≥++-+-=同理,).11(2cos 1cos ),11(2cos 1cos 322322wv w u w w C C w u v u v v B B +++-≥++++-≥+)[(21)(2122222333333222v uv u w v u w u w u w v w v v u v u w v u f +--++=++++++++-++≥∴+.21)(21)]()(2222=++=+-++-uw vw uv w uw u w vw v (取等号当且仅当w v u ==,此时,.21)],,([),21,min ======z y x f z y x c b a三、(本题满分50分)对每个正整数n ,定义函数⎪⎩⎪⎨⎧=.]}{1[,0)(不为平方数当为平方数当n n n n f(其中[x ]表示不超过x 的最大整数,]).[}{x x x -= 试求:∑=2401)(k k f 的值.解:对任意*,N k a ∈,若22)1(+<<k a k ,则k k a 212≤-≤,设,10,<<+=θθk a则].2[]}{1[,12211}{12222k a ka k a k k a k k a k a ka a -=∴+-<-+=-+=-==θθ让a 跑遍区间22)1(,(+k k )中的所有整数,则∑∑+<<==22)1(21],2[]}{1[k a k ki i ka于是∑∑∑+====2)1(1121]2[)(n a n i ki ik a f ……①下面计算∑=ki ik21],2[画一张2k×2k 的表,第i 行中,凡是i 行中的位数处填写“*”号,则这行的“*”号共]2[i k 个,全表的“*”号共∑=ki i k21]2[个;另一方面,按列收集“*”号数,第j 列中,若j 有T (j )个正因数,则该列使有T (j )个“*”号,故全表的“*”号个数共∑=kj j T 21)(个,因此∑=ki i k21]2[=∑=kj j T 21)(. 示例如下:则)]2()12([)]4()3()[1()]2()1([)()(1121n T n T T T n T T n j T a f ni ni kj +-+++-++==∑∑∑===……②由此,∑∑==+--=1512561)]()12()[16()(k k k T k T k k f ……③记,15,,2,1),2()12( =+-=k k T k T a k 易得k a 的取值情况如下:因此,∑∑===-=151161783)16()(k kk ak k f n……④据定义0)16()256(2==f f ,又当)3016(15},255,,242,241{2≤≤+=∈r rk k 设 ,301515311515151515222rr r r r rr k <++<⋅++=-+=-,231}15{13012<<+<≤r r r ,则}255,,242,241{,1]}{1[∈=k k ……⑤从则.76815783)(783)(25612401=-=-=∑∑==i i k f k f。
2006滑铁卢竞赛试题答案

Canadian Mathematics Competition
An activity of the Centre for Education in Mathematics and Computing, University of Waterloo, Waterloo, Ontario
Since the slopes of y = x (slope of 1) and y = −x (slope of −1) are negative reciprocals, then these lines are perpendicular, so ∠AOB = 90◦ .√ √ √ 2. Since B has coordinates (4, 4), then OB has length 42 + 42 = 32 = 4 √ √ 2 2 Since A has coordinates (−12, 12), then OA has length (−12) + 12 = 288 = 12 2. Using the Pythagorean Theorem on triangle AOB , √ √ √ √ AB = OB 2 + OA2 = 32 + 288 = 320 = 8 5 2. (a) Answer: 9 For the average of two digits to be 5, their sum must be 10. The two-digit positive integers whose digits sum to 10 are 19, 28, 37, 46, 55, 64, 73, 82, 91, of which there are 9. (b) Answer: n = 45 Solution 1 Suppose that n has digits AB . Then n = 10A + B . A+B . The average of the digits of n is 2 Putting a decimal point between the digits of n is equivalent to dividing n by 10, so the 10A + B . resulting number is 10 So we want to determine A and B so that A+B 10A + B = 10 2 10A + B = 5(A + B ) 5A = 4B Since A and B are digits such that 5A = 4B , then A = 4 and B = 5 is the only possibility. Therefore, n = 45. (We can quickly check that the average of the digits of n is 4.5, the number obtained by putting a decimal point between the digits of n.) Solution 2 When we compute the average of two digits, the result is either an integer or a half-integer (ie. a decimal number of the form a.5). Therefore, the possible averages are 0.5, 1.0, 1.5, 2.0, 2.5, 3.0, 3.5, 4.0, 4.5, 5.0, 5.5, 6.0, 6.5, 7.0, 7.5, 8.0, 8.5, 9.0. (0.0 is not possible as an two-digit integer cannot start with 0.) From this list, the only one equal to the average of the two digits forming it is 4.5. Therefore, n = 45 (formed by removing the decimal point from 4.5). (c) Solution 1 When the average of three integers is 28, their sum is 3(28) = 84. When the average of five integers is 34, their sum is 5(34) = 170. In this case, the difference between the sum of the five integers and the sum of the three integers is s + t which must equal 170 − 84 = 86. s+t = 43. Therefore, s + t = 86 and so the average of s and t is 2 Solution 2 Suppose the first three integers are a, b and c.
Fryer滑铁卢数学竞赛(Grade 9)-数学Mathematics-2005-试题 exam

2005Fryer Contest(Grade9)Wednesday,April20,20051.(a)Two circles have the same centre C.(Circles which havethe same centre are called concentric.)The larger circlehas radius10and the smaller circle has radius6.Deter-mine the area of the ring between these two circles.(b)In the diagram,the three concentric circles have radiiof4,6and7.Which of the three regions X,Y or Z hasthe largest area?Explain how you got your answer.(c)Three concentric circles are shown.The two largest cir-cles have radii of12and13.If the area of the ringbetween the two largest circles equals the area of thesmallest circle,determine the radius of the smallest cir-cle.Explain how you got your answer.2.A game begins with a row of empty boxes.On a turn,a player can put his or her initial in1box or in2adjacent boxes.(Boxes are called adjacent if they are next to each other.)Anh and Bharati alternate turns.Whoever initials the last empty box wins the game.(a)The game begins with a row of3boxes.Anh initials the middle box.Explain why thismove guarantees him a win no matter what Bharati does.2005Fryer Contest Page2(b)Now the game begins with a row of5boxes.Suppose that the following moves haveoccurred:StartAnh’s moveBharati’s moveShow a move that Anh can make next in order to guarantee that he will win.Explainhow this move prevents Bharati from winning.(c)Again the game begins with a row of5boxes.Suppose that the following move hasoccurred.StartAnh’s moveShow the two possible moves that Bharati can make next to guarantee she wins.Explainhow each of these moves prevents Anh from winning.3.A Nakamoto triangle is a right-angled triangle with integer side lengths which are in theratio3:4:5.(For example,a triangle with side lengths9,12and15is a Nakamoto triangle.)(a)If one of the sides of a Nakamoto triangle has length28,what are the lengths of the othertwo sides?(b)Find the lengths of the sides of the Nakamoto triangle which has perimeter96.Explainhow you got your answer.(c)Determine the area of each of the Nakamoto triangles which has a side of length60.Explain how you got your answers.4.Points B,C,and D lie on a line segment AE,as shown.A B C D EThe line segment AE has4basic sub-segments AB,BC,CD,and DE,and10sub-segments: AB,AC,AD,AE,BC,BD,BE,CD,CE,and DE.The super-sum of AE is the sum of the lengths of all of its sub-segments.(a)If AB=3,BC=6,CD=9,and DE=7,determine the lengths of the10sub-segments,and calculate the super-sum of AE.(b)Explain why it is impossible for the line segment AE to have10sub-segments of lengths1,2,3,4,5,6,7,8,9,and10.(c)When the super-sum of a new line segment AJ with9basic sub-segments of lengths fromleft to right of1,12,13,14,15,16,17,18,19is calculated,the answer is45.Determine the super-sum of a line segment AP with15basic sub-segments of lengths fromleft to right of1,12,13 ,...,115.。
滑铁卢数学竞赛

滑铁卢数学竞赛滑铁卢数学竞赛是加拿大滑铁卢大学举办的一项年度数学竞赛活动。
该竞赛旨在通过一系列难度不断增加的数学问题,考察参赛者的数学思维能力、解题能力以及创造力。
每年都有来自世界各地的学生参加该比赛,其中包括来自中小学的学生以及大学生。
滑铁卢数学竞赛分为两个阶段,第一阶段为全球性选拔赛,任何人都可以参加。
参赛者需要在线完成一套由滑铁卢大学编制的数学测试,题型涵盖代数、几何、组合数学等多个数学领域。
根据第一阶段的成绩,滑铁卢大学将选拔出前几百名成绩优异的参赛者晋级到第二阶段。
第二阶段为面试阶段,只有第一阶段晋级的学生才可以参加。
参赛者需要前往滑铁卢大学进行现场的笔试和面试。
笔试部分主要考察参赛者的数学基础知识和解题能力,而面试部分则更加注重参赛者的思维过程和解题思路。
面试时,学生需要与评委进行面对面的交流,展示自己的数学思考能力。
滑铁卢数学竞赛的题目通常非常有难度,涉及到一些高级数学概念和方法。
参赛者需要具备扎实的数学基础知识,并且具备独立思考和解决问题的能力。
竞赛的目的不仅是测试学生的数学水平,更重要的是培养他们解决问题的能力和数学思维方式。
参加滑铁卢数学竞赛对于学生来说是一次宝贵的经历。
这个竞赛可以提供一个展示自己数学才能的平台,也可以锻炼参赛者的思维能力和团队合作精神。
在竞赛中,学生们可以结识来自不同国家和地区的志同道合的数学爱好者,分享彼此的数学体验和解题方法。
滑铁卢数学竞赛也为参赛者提供了一些奖励和机会。
根据参赛者在竞赛中的表现,滑铁卢大学会为他们颁发证书和奖状,并且可以获得一些奖金和奖品。
此外,优秀的参赛者还有机会获得滑铁卢大学的奖学金和入学机会,为他们的未来发展开启了一扇大门。
总之,滑铁卢数学竞赛是一个非常有挑战性和有意义的数学竞赛活动。
通过参加这个竞赛,学生们可以提升自己的数学能力,拓展自己的数学视野,同时也能够展示自己的才能和潜力。
无论是对于中小学生还是大学生,参加滑铁卢数学竞赛都是一个值得鼓励和支持的选择。
滑铁卢数学竞赛

滑铁卢数学竞赛1、21.|x|>3表示的区间是()[单选题] *A.(-∞,3)B.(-3,3)C. [-3,3]D. (-∞,-3)∪(3,+ ∞)(正确答案)2、15.下列数中,是无理数的为()[单选题] *A.-3.14B.6/11C.√3(正确答案)D.03、9.一棵树在离地5米处断裂,树顶落在离树根12米处,问树断之前有多高()[单选题] *A. 17(正确答案)B. 17.5C. 18D. 204、若tan(π-α)>0且cosα>0,则角α的终边在()[单选题] *A.第一象限B.第二象限C.第三象限D.第四象限(正确答案)5、下列说法正确的是[单选题] *A.带“+”号和带“-”号的数互为相反数B.数轴上原点两侧的两个点表示的数是相反数C.和一个点距离相等的两个点所表示的数一定互为相反数D.一个数前面添上“-”号即为原数的相反数(正确答案)6、16、在中,则( ). [单选题] *A. AB<2AC (正确答案)B. AB=2ACC. AB>2ACD. AB与2AC关系不确定7、260°是第()象限角?[单选题] *第一象限第二象限第三象限(正确答案)第四象限8、4.已知两圆的半径分别为3㎝和4㎝,两个圆的圆心距为10㎝,则两圆的位置关系是()[单选题] *A.内切B.相交C.外切D.外离(正确答案)9、下列各角中,是界限角的是()[单选题] *A. 1200°B. -1140°C. -1350°(正确答案)D. 1850°10、下列各对象可以组成集合的是()[单选题] *A、与1非常接近的全体实数B、与2非常接近的全体实数(正确答案)C、高一年级视力比较好的同学D、与无理数相差很小的全体实数11、若2?=a2=4 ?,则a?等于( ) [单选题] *A. 43B. 82C. 83(正确答案)D. 4?12、47、若△ABC≌△DEF,AB=2,AC=4,且△DEF的周长为奇数,则EF的值为()[单选题] *A.3B.4C.1或3D.3或5(正确答案)13、8. 下列事件中,不可能发生的事件是(? ? ).[单选题] *A.明天气温为30℃B.学校新调进一位女教师C.大伟身长丈八(正确答案)D.打开电视机,就看到广告14、3.中国是最早采用正负数表示相反意义的量,并进行负数运算的国家.若零上10℃记作+10℃,则零下10℃可记作()[单选题] *A.10℃B.0℃C.-10 ℃(正确答案)D.-20℃15、19.对于实数a、b、c,“a>b”是“ac2(c平方)>bc2(c平方) ; ”的()[单选题] * A.充分不必要条件B.必要不充分条件(正确答案)C.充要条件D.既不充分也不必要条件16、22.如图棋盘上有黑、白两色棋子若干,找出所有使三颗颜色相同的棋在同一直线上的直线,满足这种条件的直线共有()[单选题] *A.5条(正确答案)B.4条C.3条D.2条17、f(x)=-2x+5在x=1处的函数值为()[单选题] *A、-3B、-4C、5D、3(正确答案)18、10. 如图所示,小明周末到外婆家,走到十字路口处,记不清哪条路通往外婆家,那么他一次选对路的概率是(? ? ?).[单选题] *A.1/2B.1/3(正确答案)C.1/4D.119、15.如图所示,下列数轴的画法正确的是()[单选题] *A.B.C.(正确答案)D.20、-950°是()[单选题] *A. 第一象限角B. 第二象限角(正确答案)C. 第三象限角D. 第四象限角21、1.如图,∠AOB=120°,∠AOC=∠BOC,OM平分∠BOC,则∠AOM的度数为()[单选题] *A.45°B.65°C.75°(正确答案)D.80°22、27.下列计算正确的是()[单选题] *A.(﹣a3)2=a6(正确答案)B.3a+2b=5abC.a6÷a3=a2D.(a+b)2=a2+b223、16.“x2(x平方)-4x-5=0”是“x=5”的( ) [单选题] *A.充分不必要条件B.必要不充分条件(正确答案)C.充要条件D.既不充分也不必要条件24、19.下列两个数互为相反数的是()[单选题] *A.(﹣)和﹣(﹣)B.﹣5和(正确答案)C.π和﹣14D.+20和﹣(﹣20)25、13.在数轴上,下列四个数中离原点最近的数是()[单选题] *A.﹣4(正确答案)B.3C.﹣2D.626、14.命题“?x∈R,?n∈N*,使得n≥x2(x平方)”的否定形式是()[单选题] * A.?x∈R,?n∈N*,使得n<x2B.?x∈R,?x∈N*,使得n<x2C.?x∈R,?n∈N*,使得n<x2D.?x∈R,?n∈N*,使得n<x2(正确答案)27、8.(2020·课标Ⅱ)已知集合U={-2,-1,0,1,2,3},A={-1,0,1},B={1,2},则?U(A∪B)=( ) [单选题] *A.{-2,3}(正确答案)B.{-2,2,3}C.{-2,-1,0,3}D.{-2,-1,0,2,3}28、7人小组选出2名同学作正副组长,共有选法()种。
2005年全国高中数学联合竞赛试题及解答.

2005年全国高中数学联合竞赛一试一、选择题:本大题共6个小题,每小题6分,共36分。
2005*1、使关于x 的不等式k x x ≥-+-63有解得实数k 的最大值为A.36- B.3C.36+ D.6◆答案:D ★解析:令=y x x -+-63,63≤≤x,可得62≤y,即6max =y,所以6≤k 2005*2、空间四点D C B A ,,,3=7=11=9=,则BD AC ⋅的取值A.只有一个B.有二个C.有四个D.有无穷多个◆答案:A★解析:注意到,9711301132222+==+由于,0 =+++则22DA DA ==-=⋅+⋅+⋅+++=++22222)(2)(AB AB CD CD BC BC AB CD BC AB CD BC AB +++-=⋅+⋅+⋅+++CD BC AB BC CD BC (2)(2222222),()CD BC BC +⋅即,022222=--+=⋅CD AB BC AD BD AC ⋅∴只有一个值为0,故选A。
2005*3、ABC ∆内接于单位圆,三个内角C B A ,,的平分线延长后分别交此圆于111,,C B A .则CB AC CC B BB A AA sin sin sin 2cos 2cos 2cos111++++的值为A.2B.4C.6D.8◆答案:A★解析:如图,连1BA ,则12sin()2sin()2222A A B C B C AA B ++=+=+-2cos().22B C =-所以B C B C A C B A A C B A AA sin sin 2cos 2cos 2cos 22cos 22cos 1+=-++-+=⎪⎭⎫⎝⎛-=,C A B BB sin sin 2cos 1+=,B A CCC sin sin 2cos 1+=。
所以()C B A CCC B BB A AA sin sin sin 22cos 2cos 2cos 111++=++,即可求得。
2005年中国第二届东南地区数学奥林匹克竞赛试题及解答(2005年7月10日)
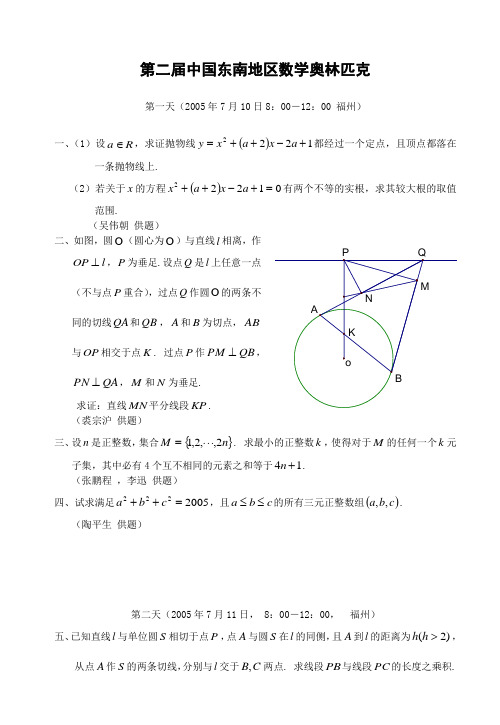
第二届中国东南地区数学奥林匹克第一天(2005年7月10日8:00-12:00 福州)一、(1)设R a ∈,求证抛物线()1222+-++=a x a x y 都经过一个定点,且顶点都落在一条抛物线上.(2)若关于x 的方程()01222=+-++a x a x 有两个不等的实根,求其较大根的取值范围.(吴伟朝 供题)二、如图,圆O (圆心为O )与直线l 相离,作l OP ⊥,P 为垂足.设点Q 是l 上任意一点(不与点P 重合),过点Q 作圆O 的两条不同的切线QA 和QB ,A 和B 为切点,AB与OP 相交于点K . 过点P 作QB PM ⊥,QA PN ⊥,M 和N 为垂足.求证:直线MN 平分线段KP .(裘宗沪 供题)三、设n 是正整数,集合{}n M 2,,2,1⋅⋅⋅=. 求最小的正整数k ,使得对于M 的任何一个k 元子集,其中必有4个互不相同的元素之和等于14+n .(张鹏程 ,李迅 供题)四、试求满足2005222=++c b a ,且c b a ≤≤的所有三元正整数组()c b a ,,.(陶平生 供题)第二天(2005年7月11日, 8:00-12:00, 福州)五、已知直线l 与单位圆S 相切于点P ,点A 与圆S 在l 的同侧,且A 到l 的距离为)2(>h h ,从点A 作S 的两条切线,分别与l 交于C B ,两点. 求线段PB 与线段PC 的长度之乘积.(冷岗松 ,司林 供题)六、将数集},...,,{21n a a a A =中所有元素的算术平均值记为)(A P ,(na a a A P n +++=...)(21). 若B 是A 的非空子集,且)()(A P B P =,则称B 是A 的一个“均衡子集”. 试求数集}9,8,7,6,5,4,3,2,1{=M 的所有“均衡子集”的个数.(陶平生 供题)七、(1)讨论关于x 的方程a x x x =+++++|3||2||1|的根的个数.(2)设n a a a ,...,,21为等差数列,且507222*********=-+⋅⋅⋅+-+-=++⋅⋅⋅++++=+⋅⋅⋅++n n n a a a a a a a a a 求项数n 的最大值.(林常 供题) 八、设2,,0πγβα<<,且1sin sin sin 333=++γβα, 求证:.233tan tan tan 222≥++γβα (李胜宏 供题)。
2005AMC12B解答

y P
M
N
6
J6
K 6 6L
O
x
15. (D) The sum of the digits 1 through 9 is 45, so the sum of the eight digits is between 36 and 44, inclusive. The sum of the four units digits is between 1 + 2 + 3 + 4 = 10 and 6 + 7 + 8 + 9 = 30, inclusive, and also ends in 1. Therefore the sum of the units digits is either 11 or 21. If the sum of the units digits is 11, then the sum of the tens digits is 21, so the sum of all eight digits is 32, an impossibility. If the sum of the units digits is 21, then the sum of the tens digits is 20, so the sum of all eight digits is 41. Thus the missing digit is 45 − 41 = 4. Note that the numbers 13, 25, 86, and 97 sum to 221.
OR
Each of the two-digit numbers leaves the same remainder when divided by 9 as does the sum of its digits. Therefore the sum of the four two-digit numbers leaves the same remainder when divided by 9 as the sum of all eight digits. Let d be the missing digit. Because 221 when divided by 9 leaves a remainder of 5, and the sum of the digits from 1 through 9 is 45, the number (45 − d) must leave a remainder of 5 when divided by 9. Thus d = 4.
05历届国际物理奥林匹克竞赛试题与解答

历届国际物理奥林匹克竞赛试题与解答第5届(1971年于保加利亚的索菲亚)【题1】质量为m 1和m 2的物体挂在绳子的两端,绳子跨过双斜面顶部的滑轮,如图5.1。
斜面质量为m ,与水平面的夹角为α 1和α 2。
整个系统初态静止。
求放开后斜面的加速度和物体的加速度。
斜面保持静止的条件是什么?摩擦可以忽略。
解:我们用a 表示双斜面在惯性参照系中的加速度(正号表示向右的方向)。
用a 0表示物体相对斜面的加速度(正号表示左边物体m 1下降)两个物体在惯性系中的加速度a 1和a 2可由矢量a 和a 0相加得到(如解 图5.1 图5.1)。
用F 表示绳子中的张力。
对沿斜面方向的分量应用牛顿第二定律。
使物体m 1加速下降的力是 m 1g sin α 1-F在惯性系中,沿斜面方向的加速度分量为 a 0-a cos α 1所以,对此斜面分量,牛顿第二定律为: 解图5.1 m 1(a 0-a cos α 1)=m 1g sin α 1-F 同样,对于m 2有m 2(a 0-a cos α 2)=F -m 2g sin α 2 两式相加:(m 1cos α 1+m 2cos α 2)a =(m 1+m 2)a 0-(m 1sin α 1-m 2sin α 2)g (1)我们用动量守恒原理来研究斜面的运动。
斜面在惯性系中的速度为v (向右)。
物体相对斜面的速度为v 0。
故斜面上两物体在惯性系中的速度的水平分量(向左)分别为: v 0 cos α 1-v 和 v 0 cos α 2-v 利用动量守恒原理:m 1(v 0 cos α 1-v )+m 2(v 0 cos α 2-v )=m v 对匀加速运动,速度与加速度成正比,因此有:m 1(a 0 cos α 1-a )+m 2(a 0 cos α 2-a )=m a所以0212211cos cos a m m m m m a +++=αα (2)上式给出了有关加速度的信息。
2005联赛答案
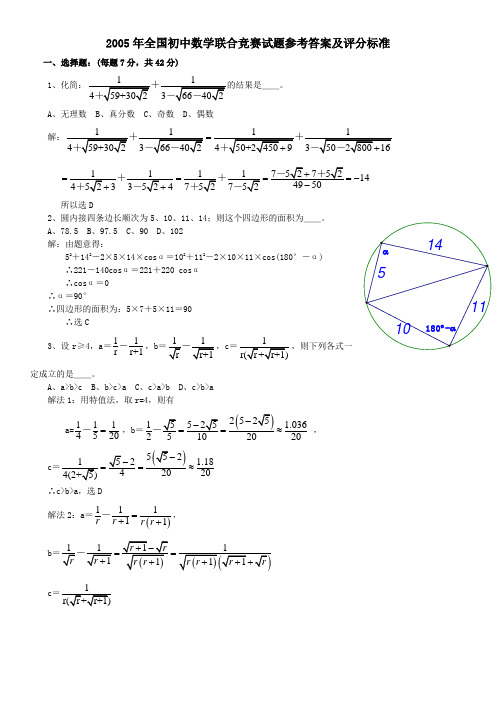
2005年全国初中数学联合竞赛试题参考答案及评分标准一、选择题:(每题7分,共42分)1、化简:11459+302366402++--的结果是__。
A 、无理数B 、真分数C 、奇数D 、偶数 解:1111459+302366402450+24509350280016=+++++--+--111175275214495045233524752752+====--++-++++-+-所以选D2、圆内接四条边长顺次为5、10、11、14;则这个四边形的面积为__。
A 、78.5 B 、97.5 C 、90 D 、102 解:由题意得:52+142-2×5×14×cos α=102+112-2×10×11×cos(180°-α) ∴221-140cos α=221+220 cos α ∴cos α=0 ∴α=90°∴四边形的面积为:5×7+5×11=90 ∴选C3、设r ≥4,a =11rr+1-,b =11r r+1-,c =1r(r +r+1),则下列各式一定成立的是__。
A 、a>b>cB 、b>c>aC 、c>a>bD 、c>b>a 解法1:用特值法,取r=4,则有a=1114520=-,b =()252515525 1.03625102020--==≈- , c =()552152 1.18420204(2+5)--==≈∴c>b>a ,选D 解法2:a =()11111rr r r =++-, b =()()()11111111r r r r r r r r r r+-==+++++- c =1r(r +r+1)5101114180︒-αα()()()()()()()()()()()()()()()()()()()()4,11111111111111,111110111,:,Dr r r r r r rr r r r r rr r r r r r r r r r a br r r r r r rr rr r r r r r r r rrb c a b c≥∴+-+++⎡⎤=++-+-⎣⎦⎡⎤=+-+-->⎣⎦∴+>+++<+++-++=+++->∴+++>++<<< 故又 故综上所述选 解法3:∵r ≥ 4 ∴111r r ++<1∴111111111a b rr r r r r ⎛⎫⎛⎫=+-<-=⎪⎪+++⎝⎭⎝⎭c =111111r r r r b r r r r r +-+->=-=++∴a<b<c ,选D4、图中的三块阴影部分由两个半径为1的圆及其外公切线分割而成,如果中间一块阴影的面积等于上下两块面积之和,则这两圆的公共弦长是__。
2005年全国高中数学联赛试卷及解答

2005年全国高中数学联赛试卷(2005年10月16日上午8∶00-9∶40)一、选择题:1.使关于x 的不等式x -3+6-x ≥k 有解的实数k 的最大值是 ( ) A .6- 3 B . 3 C .6+ 3 D . 62.空间四点A 、B 、C 、D 满足|→AB |=3,|→BC |=7,|→CD |=11,|→DA |=9.则→AC ·→BD 的取值( ) A .只有一个 B .有二个 C .有四个 D .有无穷多个3.△ABC 内接于单位圆,三个内角A 、B 、C 的平分线延长后分别交此圆于A 1、B 1、C 1,则AA 1·cos A 2+BB 1·cos B 2+CC 1·cosC2sin A +sin B +sin C 的值为 ( )A .2B .4C .6D .84.如图,ABCD -A 'B 'C 'D '为正方体,任作平面α与对角线AC '垂直,使得α与正方体的每个面都有公共点,记这样得到的截面多边形的面积为S ,周长为l ,则 ( ) A .S 为定值,l 不为定值 B .S 不为定值,l 为定值 C .S 与l 均为定值 D .S 与l 均不为定值5.方程x 2sin 2-sin 3+y 2cos 2-cos 3=1表示的曲线是 ( )A .焦点在x 轴上的椭圆B .焦点在x 轴上的双曲线C .焦点在y 轴上的椭圆D .焦点在y 轴上的双曲线6.记集合T ={0,1,2,3,4,5,6},M ={a 17+a 272+a 373+a 474| a i ∈T ,i =1,2,3,4},将M 中的元素按从大到小排列,则第2005个数是 ( )A .57+572+673+374B .57+572+673+274C .17+172+073+474D .17+172+073+374二、填空题:7.将关于x 的多项式f (x )=1-x +x 2-x 3+…-x 19 +x 20表为关于y 的多项式g (y )=a 0+a 1y +a 2y 2+…+a 19y 19+a 20y 20,其中y =x -4,则a 0+a 1+…+a 20= ;8.已知f (x )是定义在(0,+∞)上的减函数,若f (2a 2+a +1)<f (3a 2-4a +1)成立,则a 的取值范围是 ;9.设α、β、γ满足0<α<β<γ<2π,若对于任意x ∈R ,cos(x +α)+cos(x +β)+cos(x +γ)=0,则γ-α= ;10.如图,四面体DABC 的体积为16,且满足∠ACB =45︒,AD +BC +AC 2=3,则CD = ;11.若正方形ABCD 的一条边在直线y =2x -17上,另外两个顶点在抛物线y =x 2上,则该正方形面积的最小值为 ;12.如果自然数a 的各位数字之和等于7,那么称a 为“吉祥数”.将所有“吉祥数”从小到大排成一列a 1,a 2,a 3,…,若a n =2005,则a 5n = .三、解答题:A'B'C'D'D C BA 45°AD CB证明:⑴ 对任意n ∈N ,a n 为正整数;⑵ 对任意n ∈N ,a n a n +1-1为完全平方数.14.将编号为1,2,3,…,9的九个小球随机放置在圆周的九个等分点上,每个等分点上各放一个小球,设圆周上所有相邻两个球号码之差的绝对值之和为S ,求使S 达到最小值的放法的概率.(注:如果某种放法,经旋转或镜面反射后与另一种放法重合,则认为是相同的放法)15.过抛物线y =x 2上一点A (1,1)作抛物线的切线,分别交x 轴于点D ,交y 轴于点B ,点C 在抛物线上,点E 在线段AC 上,满足AE EC =λ1;点F 在线段BC 上,满足BFFC =λ2,且λ1+λ2=1,线段CD 与EF 交于点P ,当点C 在抛物线上移动时,求点P 的轨迹方程.加试卷一、如图,在△ABC 中,设AB >AC ,过点A 作△ABC 的外接圆的切线l ,又以点A 为圆心,AC 为半径作圆分别交线段AB 于点D ;交直线l 于点E 、F .证明:直线DE 、DF 分别通过△ABC 的内心与一个旁心.二、设正数a 、b 、c 、x 、y 、z 满足cy +bz =a ,az +cx =b ,bx +ay =c .求函数f (x ,y ,z )=x 21+x +y 21+y +z 21+z的最小值.三、对每个正整数n ,定义函数f (n )=⎩⎪⎨⎪⎧0,当n 为完全平方数,[1{n }],当n 不为完全平方数.(其中[x ]表示不超过x 的最大整数,{x }=x -[x ]).试求k =1∑240f (k )的值.呜呼!不怕繁死人,就怕繁不成!2005年全国高中数学联赛试卷(2005年10月16日上午8∶00-9∶40)一、选择题:1.使关于x 的不等式x -3+6-x ≥k 有解的实数k 的最大值是 ( ) A .6- 3 B . 3 C .6+ 3 D . 6 选D .解:3≤x ≤6,令x -3=3sin α(0≤α≤π2),则x =3+3sin 2α,6-x =3cos α.故6≥3(sin α+cos α)≥3.故选D .2.空间四点A 、B 、C 、D 满足|→AB |=3,|→BC |=7,|→CD |=11,|→DA |=9.则→AC ·→BD 的取值( ) A .只有一个 B .有二个 C .有四个 D .有无穷多个 选A .解:→AB +→BC +→CD +→DA =→0.DA 2=→DA 2=(→AB +→BC +→CD )2=AB 2+BC 2+CD 2+2(→AB ·→BC +→AB ·→CD +→BC ·→CD )=AB 2+BC 2+CD 2+2(→AB ·→BD +→BC ·→BD -→BC 2),(其中→BC +→CD =→BD ,→CD =→BD -→BC ) =AB 2+BC 2+CD 2-2BC 2+2(→AC ·→BD ).故2→AC ·→BD =DA 2+BC 2-AB 2-CD 2=92+72-32-112=0⇒→AC ·→BD =0.选A .3.△ABC 内接于单位圆,三个内角A 、B 、C 的平分线延长后分别交此圆于A 1、B 1、C 1,则AA 1·cos A 2+BB 1·cos B 2+CC 1·cosC2sin A +sin B +sin C的值为 ( )A .2B .4C .6D .8 选A .解:AA 1·cos A 2=2sin(B +A 2)cos A2=sin(A +B )+sin B =sin C +sin B .AA 1·cos A 2+BB 1·cos B 2+CC 1·cos C2=2(sin A +sin B +sin C ).故原式=2.选A .4.如图,ABCD -A 'B 'C 'D '为正方体,任作平面α与对角线AC '垂直,使得α与正方体的每个面都有公共点,记这样得到的截面多边形的面积为S ,周长为l ,则 ( )A .S 为定值,l 不为定值B .S 不为定值,l 为定值C .S 与l 均为定值D .S 与l 均不为定值 选B .解:设截面在底面内的射影为EFBGHD ,设AB =1,AE =x (0≤x ≤12),则l =3[2x +2(1-x )]=32为定值;而S =[1-12x 2-12(1-x )2]secθ=(12-x -x 2)secθ(θ为平面α与底面的所成角)不为定值.故选B .ACBA 1B 1C 1IE FGHA'B'C'D'D CB A5.方程x 2sin 2-sin 3+y 2cos 2-cos 3=1表示的曲线是 ( )A .焦点在x 轴上的椭圆B .焦点在x 轴上的双曲线C .焦点在y 轴上的椭圆D .焦点在y 轴上的双曲线 选C .解:由于3+2>π⇒π2>3-π2>π2-2>0⇒cos(3-π2)<cos(π2-2)⇒sin 2-sin 3>0;又,0<2<3c <π⇒cos 2-cos 3>0,⇒曲线为椭圆.sin 2-sin 3-(cos 2-cos 3)=2[sin(2-π4)-sin(3-π4)].而0<2-π4<3-π4<π2⇒sin 2-sin 3<cos 2-cos 3⇒焦点在y 轴上.故选C .6.记集合T ={0,1,2,3,4,5,6},M ={a 17+a 272+a 373+a 474| a i ∈T ,i =1,2,3,4},将M 中的元素按从大到小排列,则第2005个数是 ( )A .57+572+673+374B .57+572+673+274C .17+172+073+474D .17+172+073+374选C .解:M ={174(a 1×73+a 2×72+a 3×7+a 4)| a i ∈T ,i =1,2,3,4},a 1×73+a 2×72+a 3×7+a 4可以看成是7进制数,(a 1a 2a 3a 4)7,其最大的数为(6666)7=74-1=2400.从而从大到小排列的第2005个数是2400-2004=396,即从1起从小到大排的第396个数,396=73+72+4⇒(1104)7,故原数为17+172+073+474.故选C .二、填空题:7.将关于x 的多项式f (x )=1-x +x 2-x 3+…-x 19 +x 20表为关于y 的多项式g (y )=a 0+a 1y +a 2y 2+…+a 19y 19+a 20y 20,其中y =x -4,则a 0+a 1+…+a 20= ;填521+16解:f (x )=a 0+a 1(x -4)2+a 2(x -4)2+…+a 20(x -4)20.令x =5得f (5)=1-5+52-53+…-519+520=(-5)21-1(-5)-1=521+16=a 0+a 1+…+a 20.8.已知f (x )是定义在(0,+∞)上的减函数,若f (2a 2+a +1)<f (3a 2-4a +1)成立,则a 的取值范围是 ;填(0,13)∪(1,5).解:⎩⎨⎧2a 2+a +1>0,3a 2-4a +1>0.⇒a ∈(-∞,13)∪(1,+∞).2a 2+a +1>3a 2-4a +1⇒a 2-5a <0⇒0<a <5. 故所求取值范围为(0,13)∪(1,5).9.设α、β、γ满足0<α<β<γ<2π,若对于任意x ∈R ,cos(x +α)+cos(x +β)+cos(x +γ)=0,则γ-α= ;填43π. 解:由f (x )≡0,得f (-α)=f (-β)=f (-γ)=0:cos (β-α)+cos(γ-α)=cos(β-α)+cos(γ-β)=cos(γ-α)+cos(γ-β)=-1. 故cos(β-α)=cos(γ-β)=cos(γ-α)=-12,由于0<α<β<γ<2π,故β-α,γ-β,γ-α∈{23π,43π}.从而γ-α=43π.10.如图,四面体DABC 的体积为16,且满足∠ACB =45︒,AD +BC +AC2=3,则CD = ;填3.解:V =13×12AC ×BC sin45︒×h ≤16AC ×BC ×AD sin45︒.即AC ×BC ×AD sin45︒≥1⇒AC2×BC ×AD ≥1.而3=AD +BC +AC2≥33AD ·BC ·AD 2=3,等号当且仅当AD =BC =AC2=1时成立,故AC =2,且AD =BC =1,AD ⊥面ABC .⇒CD =3.11.若正方形ABCD 的一条边在直线y =2x -17上,另外两个顶点在抛物线y =x 2上,则该正方形面积的最小值为 ;填80.解:设正方形ABCD 的顶点A 、B 在抛物线上,C 、D 在直线上. 设直线AB 方程为y =2x +b , ⑴ 求AB 交抛物线y =x 2的弦长:以y =2x +b 代入y =x 2,得x 2-2x -b =0.△=4+4b ⇒l =25(b +1).⑵ 两直线的距离=|b +17|5.⑶ 由ABCD 为正方形得,25(b +1)=|b +17|5⇒100(b +1)=b 2+34b +289⇒b 2-66b +189=0.解得b =3,b =63.正方形边长=45或165⇒正方形面积最小值=80.12.如果自然数a 的各位数字之和等于7,那么称a 为“吉祥数”.将所有“吉祥数”从小到大排成一列a 1,a 2,a 3,…,若a n =2005,则a 5n = .填52000.解:一位的吉祥数有7,共1个;二位的吉祥数有16,25,34,43,52,61,70,共7个;三位的吉祥数为x 1+x 2+x 3=7的满足x 1≥1的非负整数解数,有C 82=28个(也可枚举计数).一般的,k 位的吉祥数为x 1+x 2+…+x k =7的满足x 1≥1的非负整数解数,令x i '=x i +1(i =2,3,…,k ),有x 1+x 2'+…+x k '=7+k -1.共有解C k +5k -1=C k +56组.4位吉祥数中首位为1的有28个,2005是4位吉祥数中的第29个.故n =1+7+28+28+1=65.5n =325.C 66+C 76+C 86+C 96+C 106=1+7+28+84+210=330.即是5位吉祥数的倒数第6个: 5位吉祥数从大到小排列:70000,61000,60100,60010,60001,52000,….45°ADCB三、解答题:13.数列{a n }满足a 0=1,a n +1=7a n +45a n 2-362,n ∈N ,证明:⑴ 对任意n ∈N ,a n 为正整数;⑵ 对任意n ∈N ,a n a n +1-1为完全平方数. 证明:⑴ a 1=5,且a n 单调递增.所给式即 (2a n +1-7a n )2=45a n 2-36⇒a n +12 -7a n +1a n +a n 2+9=0. ①下标加1: a n +22 -7a n +2a n +1+a n +12+9=0. ②相减得: (a n +2-a n )(a n +2-7a n +1+a n )=0.由a n 单调增,故a n +2-7a n +1+a n =0⇒a n +2=7a n +1-a n . ③因a 0、a 1为正整数,且a 1>a 0,故a 2为正整数,由数学归纳法,可知,对任意n ∈N ,a n 为正整数. ⑵ 由①:a n +12 +2a n +1a n +a n 2=9(a n +1a n -1)⇒a n +1a n -1=(a n +a n +13)2 ④ 由于a n 为正整数,故a n +1a n -1为正整数,从而(a n +a n +13)2为正整数.但a n 、a n +1均为正整数,于是a n +a n +13必为有理数,而有理数的平方为整数时,该有理数必为整数,从而a n +a n +13是整数.即a n +1a n -1是整数的平方,即为完全平方数.故证.原解答上有一段似无必要:记f (n )=a n +1a n -(a n +a n +13)2,则f (n )-f (n -1)=(a n +1a n -a n a n -1)-19(2a n +a n +1+a n -1)(a n +1-a n -1)=19(a n -1-a n +1)(a n +1-7a n +a n -1)=0.即f (n )=f (n -1)=…=f (0)=1,故④式成立.故a n a n +1-1为完全平方数.又证:由上证,得③式后:a n +2-7a n +1+a n =0. 特征方程为 x 2-7x +1=0.解得: x =7±352=⎝ ⎛⎭⎪⎫3±522=⎝ ⎛⎭⎪⎫5±124. 令 a n =α⎝⎛⎭⎫5+124n +β⎝⎛⎭⎪⎫5-124n .由a 0=1,a 1=5解得α=5+125,β=5-125; 得 a n =15[⎝⎛⎭⎫5+124n +1+⎝⎛⎭⎪⎫5-124n +1] ⑤ 注意到5+12·5-12=1,5+12+5-12=5. 有, a n a n +1-1=15[⎝ ⎛⎭⎪⎫5-124n +1+⎝⎛⎭⎫5+124n +1]·[⎝⎛⎭⎫5+124n +5+⎝ ⎛⎭⎪⎫5-124n +5]-1 =15[⎝⎛⎭⎫5+128n +6+⎝ ⎛⎭⎪⎫5-128n +6+⎝⎛⎭⎫5+124+⎝⎛⎭⎫5+124-5] =15[⎝⎛⎭⎫5+124n +3+⎝ ⎛⎭⎪⎫5-124n +3]2 由二项式定理或数学归纳法知⎝⎛⎭⎫5+124n +3+⎝ ⎛⎭⎪⎫5-124n +3为k 5型数(k ∈N *),故a n a n +1-1为完全平方数. (用数学归纳法证明:n =0时,⎝⎛⎭⎫5+123+⎝⎛⎭⎪⎫5-123=25.设当n ≤m (m ∈N *)时,⎝⎛⎭⎫5+124n +3+⎝ ⎛⎭⎪⎫5-124n +3=k n 5(k n ∈N *),且k 1<k 2<…<k m .⎝⎛⎭⎫5+124(m +1)+3+⎝ ⎛⎭⎪⎫5-124(m +1)+3=[⎝⎛⎭⎫5+124m +3+⎝ ⎛⎭⎪⎫5-124m +3]·[⎝⎛⎭⎫5+124+⎝ ⎛⎭⎪⎫5-124]-[⎝⎛⎭⎫5+124m -1+⎝ ⎛⎭⎪⎫5-124m -1].=7k m 5-k m -15=(7k m -k m -1)5.由归纳假设知k m +1=7k m -k m -1∈N *,且k m <k m +1成立.得证.14.将编号为1,2,3,…,9的九个小球随机放置在圆周的九个等分点上,每个等分点上各放一个小球,设圆周上所有相邻两个球号码之差的绝对值之和为S ,求使S 达到最小值的放法的概率.(注:如果某种放法,经旋转或镜面反射后与另一种放法重合,则认为是相同的放法)解:9个有编号的小球放在圆周的九个九等分点上,考虑镜面反射的因素,共有8!2种放法;为使S 取得最小值,从1到9之间应按增序排列:设从1到9之间放了k 个球,其上的数字为x 1,x 2,…,x k ,则|1-x 1|+|x 1-x 2|+…+|x k -9|≥|1-x 1+x 1-x 2+…+x k -9|=8.当且仅当1-x 1、x 1-x 2、…、x k -9全部同号时其和取得最小值,即1,x 1,x 2,…,x k ,9递增排列时其和最小.故S ≥2×8=16.当S 取得最小值时,把除1、9外的7个元素分成两个子集,各有k 及7-k 个元素,分放1到9的两段弧上,分法总数为C 70+C 71+…+C 76种,考虑镜面因素,共有64种方法.所求概率P =64×28!=1315.15.过抛物线y =x 2上一点A (1,1)作抛物线的切线,分别交x 轴于点D ,交y 轴于点B ,点C 在抛物线上,点E 在线段AC 上,满足AE EC =λ1;点F 在线段BC 上,满足BFFC =λ2,且λ1+λ2=1,线段CD 与EF 交于点P ,当点C 在抛物线上移动时,求点P 的轨迹方程.解:过点A 的切线方程为y =2x -1.交y 轴于点B (0,-1).AB 与x 轴交于点D (12,0).设点C 坐标为C (x 0,y 0),CDCP=λ,点P 坐标为(x ,y ).由AE EC =λ1⇒AC CE =1+λ1,同理,CBCF=1+λ2; 而CA CE 、CD CP 、CBCF 成等差数列(过A 、B 作CD 的平行线可证). 得2λ=1+λ1+1+λ2=3,即λ=32.从而点P 为△ABC 的重心.x =1+0+x 03,y =1+(-1)+y 03.y 0=x 02. 解得x 0=3x -1,y 0=3y ,代入y 0=x 02得,y =13(3x -1)2. 由于x 0≠1,故x ≠23.所求轨迹方程为y =13(3x -1)2(x ≠23).又解:过点A 的切线方程为y =2x -1.交y 轴于点B (0,-1).AB 与x 轴交于点D (12,0).设点C 坐标为C (t ,t 2),CD 方程为x -12t -12=y t 2,即y =t 22t -1(2x -1).点E 、F 坐标为E (1+λ1t 1+λ1,1+λ1t 21+λ1);F (λ2t 1+λ2,λ2t 2-11+λ2).从而得EF 的方程为:y -1+λ1t 21+λ1λ2t 2-11+λ2-1+λ1t21+λ1=x -1+λ1t1+λ1λ2t 1+λ2-1+λ1t 1+λ1. 化简得:[(λ2-λ1)t -(1+λ2)]y =[(λ2-λ1)t 2-3]x +1+t -λ2t 2. ① 当t ≠12时,直线CD 方程为: y =2t 2x -t 22t -1②联立①、②解得⎩⎨⎧x =t +13,y =t 23. 消去t ,得点P 的轨迹方程为y =13(3x -1)2.当t =12时,EF 方程为:-32y =(14λ2-14λ1-3)x +32-14λ2,CD 方程为:x =12,联立解得点(12,112),此点在上述点P 的轨迹上,因C 与A 不能重合,故t ≠1,x ≠23.故所求轨迹为 y =13(3x -1)2 (x ≠23).加试卷一、如图,在△ABC 中,设AB >AC ,过点A 作△ABC 的外接圆的切线l ,又以点A 为圆心,AC 为半径作圆分别交线段AB 于点D ;交直线l 于点E 、F .证明:直线DE 、DF 分别通过△ABC 的内心与一个旁心.证明:连DC 、DE ,作∠BAC 的平分线交DE 于点I ,交CD 于G . 由AD =AC ,∠DAI =∠CAI ,AI =AI ⇒△ADI ≌△ACI . 故∠ADI =∠ACI ,但∠F AD =∠ACB (弦切角);∠F AD =2∠ADE (等腰三角形顶角的外角) 所以∠F AD =2∠ACI ⇒∠ACB =2∠ACI ,即CI 是∠ACB 的平分线.故点I 是△ABC 的内心. 连FD 并延长交AI 延长线于点I ',连CI '. 由于AD =AE =AF ⇒∠EDF =90︒⇒∠IDI '=90︒.而由△ADI ≌△ACI 知,∠AID =∠AIC ⇒∠DII '=∠CII ',又ID =IC ,II '为公共边.故△IDI '≌△ICI ',⇒∠ICI '=90︒.由于CI 是∠ACB 的平分线,故CI '是其外角的平分线,从而I '为△ABC 的一个旁心.又证:⑴ 连DE 、DC ,作∠BAC 的平分线分别交DE 于I ,DC 于G ,连IC ,则由AD =AC ,得AG ⊥DC ,ID =IC .又D 、C 、E 在⊙A 上,故∠IAC =12∠DAC =∠IEC .故A 、I 、C 、E 四点共圆.所以∠CIE =∠CAE =∠ABC ,而∠CIE =2∠ICD ,故∠ICD =12∠ABC .所以,∠AIC =∠IGC +∠ICG =90︒+12∠ABC ,所以∠ACI =12∠ACB .故I 为△ABC 的内心.⑵ 连FD 并延长交∠ABC 的外角平分线于I 1,连II 1,BI 1、BI ,则由⑴知,I 为△ABC 的内心,故∠IBI 1=90︒=∠EDI 1.故D 、B 、I 1、I 四点共圆.故∠BII 1=∠BDI 1=90︒-∠ADI =(12∠BAC +∠ADG )-∠ADI =12∠BAC +∠IDG ,故A 、I 、I 1共线.所以,I 1是△ABC 的BC 边外的旁心.二、设正数a 、b 、c 、x 、y 、z 满足cy +bz =a ,az +cx =b ,bx +ay =c .求函数f (x ,y ,z )=x 21+x +y 21+y +z 21+z 的最小值.解:解方程组:⎩⎪⎨⎪⎧cy +bz =a ,az +cx =b ,bx +ay =c .得,⎩⎪⎨⎪⎧x =b 2+c 2-a 22bc,y =c 2+a 2-b22ac,z =a 2+b 2-c 22ab.由于x 、y 、z 为正数,故⎩⎪⎨⎪⎧a 2+b 2>c 2,b 2+c 2>a 2,c 2+a 2=b 2.⇒⎩⎪⎨⎪⎧a +b >c ,b +c >a ,c +a =b .即以a 、b 、c 为边可以构成锐角三角形.记边a 、b 、c 的对角分别为∠A 、∠B 、∠C .则cos A =x ,cos B =y ,cos C =z .(A 、B 、C 为锐角)f (x ,y ,z )=f (cos A ,cos B ,cos C )=cos 2A 1+cos A +cos 2B 1+cos B +cos 2C1+cos C.令u =cot A ,v =cot B ,w =cot C ,则u ,v ,w ∈R +,且uv +vw +wu =1.于是,(u +v )(u +w )=u 2+uv +uw +vw =u 2+1.同理,v 2+1=(v +u )(v +w ),w 2+1=(w +u )(w +v ). cos 2A =sin 2A cot 2A =cot 2A 1+cot 2A =u 21+u 2,所以,cos 2A 1+cos A =u 21+u 21+u 1+u 2=u 21+u 2(1+u 2+u )=u 2(1+u 2-u )1+u 2=u 2-u 31+u 2=u 2-u 3(u +v )(u +w )≥u 2-u 32(1u +v +1u +w ). 同理cos 2B 1+cos B ≥v 2-v 32(1v +u +1v +w ),cos 2C 1+cos C ≥w 2-w 32(1w +u +1w +v).于是f ≥u 2+v 2+w 2-12(u 3+v 3u +v +v 3+w 3v +w +w 3+u 3w +u)=u 2+v 2+w 2-12(u 2-uv +v 2+v 2-vw +w 2+w 2-wu +u 2)=12(uv +vw +wu )=12(等号当且仅当u =v =w ,即a =b =c ,x =y =z =12时成立.) 故知[f (x ,y ,z )]min =12.又证:由约束条件可知⎩⎪⎨⎪⎧x =b 2+c 2-a 22bc ,y =a 2+c 2-b 22ac ,z =a 2+b 2-c 22ab.故⎩⎪⎨⎪⎧1+x =(a +b +c )(-a +b +c )2bc,1+y =(a +b +c )(a -b +c )2ac,1+z =(a +b +c )(a +b -c )2ab.得,f (x ,y ,z )=12(a +b +c )⎣⎢⎡⎦⎥⎤(b 2+c 2-a 2)2bc (b +c -a )+(c 2+a 2-b 2)2ac (c +a -b ) +(a 2+b 2-c 2)2ab (a +b -c ). ⑴ 显然有a +b -c >0,a -b +c >0,-a +b +c >0.由Cauchy 不等式有,⎣⎢⎡⎦⎥⎤(b 2+c 2-a 2)2bc (b +c -a )+(c 2+a 2-b 2)2ac (c +a -b ) +(a 2+b 2-c 2)2ab (a +b -c )·[bc (b +c -a )+ca (c +a -b )+ab (a +b -c )]≥(a 2+b 2+c 2)2. 故f (x ,y ,z )≥(a 2+b 2+c 2)22(a +b +c )(b 2c +bc 2+ac 2+a 2c +a 2b +ab 2-3abc )=12·a 4+b 4+c 4+2a 2b 2+2b 2c 2+2a 2c 2 2a 2b 2+2b 2c 2+2c 2a 2+b 3c +b 3c +a 3b +a 3c +c 3a +c 3b -abc (a +b +c ). 下面证明a 4+b 4+c 4+2a 2b 2+2b 2c 2+2a 2c 22a 2b 2+2b 2c 2+2c 2a 2+b 3c +b 3c +a 3b +a 3c +c 3a +c 3b -abc (a +b +c )≥1.即证a 4+b 4+c 4≥a 3b +a 3c +b 3c +b 3a +c 3a +c 3b -(a +b +c )abc . ⑵由于,a 4-a 3b -a 3c +a 2bc =a 2(a 2-ab -ac -bc )=a 2(a -b )(a -c ).故⑵式即a 2(a -b )(a -c )+b 2(b -a )(b -c )+c 2(c -a )(c -b )≥0.不妨设a ≥b ≥c .则a 2(a -b )(a -c )+b 2(b -a )(b -c )≥a 2(a -b )(b -c )-b 2(a -b )(b -c )=(a 2-b 2)(a -b )(b -c )≥0,又,c 2(c -a )(c -b )≥0于是a 2(a -b )(a -c )+b 2(b -a )(b -c )+ c 2(c -a )(c -b )≥0成立.等号当且仅当a =b =c 时成立. 所以,f (x ,y ,z )≥12,且f (12,12,12)=12.又证:令p =12(a +b +c ),⑴式即f (x ,y ,z )=18p ⎣⎢⎡⎦⎥⎤(b 2+c 2-a 2)2bc (p -a )+(c 2+a 2-b 2)2ac (p -b ) +(a 2+b 2-c 2)2ab (p -c )(由Cauchy 不等式)≥18p ·(a 2+b 2+c 2)2bc (p -a )+ca (p -b )+ab (p -c )=18p ·(a 2+b 2+c 2)2p (ab +bc +ca )-3abc .而a 2+b 2+c 2=2(p 2-4Rr -r 2),ab +bc +ca =p 2+4Rr +r 2,abc =4Rrp .(*)故,f (x ,y ,z )≥12p ·(p 2-4Rr -r 2)2p (p 2+4Rr +r 2)-12pRr =12p 2·(p 2-4Rr -r 2)2p 2-8Rr +r 2.而(p 2-4Rr -r 2)2p 2-8Rr +r 2≥p 2⇔p 4+16R 2r 2+r 4-8p 2Rr -2p 2r 2+8Rr 3≥p 4-8p 2Rr +p 2r 2 ⇔16R 2+8Rr +r 2≥3p 2⇔4R +r ≥3p . (**) 最后一式成立.故得结论.关于(*)式:由△=rp ,得 r 2=△2p 2=p (p -a )(p -b )(p -c )p 2=(p -a )(p -b )(p -c )p=p 3-(a +b +c )p 2+(ab +bc +ca )p -abc p =-p 3+(ab +bc +ca )p -abc p; ①又由△=abc 4R ,得4Rr =abcp .故4Rr +r 2=-p 2+(ab +bc +ca ).就是 ab +bc +ca =p 2+4Rr +r 2;a 2+b 2+c 2=(a +b +c )2-2(ab +bc +ca )=4p 2-2p 2-8Rr -2r 2=2(p 2-4Rr -r 2); abc =4R △=4Rrp .文案大全4R +r =4R +4R sin A 2sin B 2sin C2=4R +4R (cos A +cos B +cos C -1)=R (3+ cos A +cos B +cos C )=2R (cos 2A 2+cos 2B 2+cos 2C2).而p =R sin A +R sin B +R sin C =4R cos A 2cos B 2cos C2.故4R +r ≥3p ⇔cos 2A 2+cos 2B 2+cos 2C 2≥23cos A 2cos B 2cos C2.又cos 2A 2+cos 2B 2+cos 2C2≥33cos 2A 2cos 2B 2cos 2C2,而33cos 2A 2cos 2B 2cos 2C 2≥23cos A 2cos B 2cos C2⇔32≤3cos A 2cos B 2cos C 2⇔ cos A 2cos B 2cos C 2≥338⇔ sin A +sin B +sin C ≤3sin π3.(由琴生不等式可证)三、对每个正整数n ,定义函数f (n )=⎩⎪⎨⎪⎧0,当n 为完全平方数,[1{n }],当n 不为完全平方数.(其中[x ]表示不超过x 的最大整数,{x }=x -[x ]).试求k =1∑240f (k )的值.解:对于任意n (n 不是完全平方数),存在k ,满足k 2<n <(k +1)2,则1≤n -k 2≤2k .此时n =k +{n }.⎣⎡⎦⎤1{n }=⎣⎢⎡⎦⎥⎤1n -k =⎣⎢⎡⎦⎥⎤n +k n -k 2=⎣⎢⎡⎦⎥⎤2k +{n }n -k 2. 由于2k <2k +{n }<2k +1.故2k n -k 2<2k +{n }n -k 2<2k +1n -k 2.从而在2k n -k 2与2k +1n -k 2之间没有整数.即⎣⎢⎡⎦⎥⎤2k +{n }n -k 2=⎣⎡⎦⎤2k n -k 2.若记n -k 2=i (i =1,2,…,2k ),又240=152+15. 于是,k =1∑240f (k )=k =1∑14i =1∑2k⎣⎡⎦⎤2k i +i =1∑15⎣⎡⎦⎤2×15i .由于k <i ≤2k 时⎣⎡⎦⎤2k i =1故i =k +1∑2k⎣⎡⎦⎤2k i =k .于是 k =1∑240f (k )=k =1∑15i =1∑k⎣⎡⎦⎤2k i +k =1∑14k =(2+6+11+16+22+29+34+42+49+56+63+72+78+87+96)+105=768. 即所求值为768. 又解:为计算i =1∑2k⎣⎡⎦⎤2k i ,画一2k ×2k 的表格,在第i 行中,凡i 的倍数处填写*号,则这行的*号共有⎣⎡⎦⎤2ki 个,全表共有i =1∑2k⎣⎡⎦⎤2k i 个.另一方面,第j 列中的*号个数等于j 的约数的个数T (j ),从而全表中的*号个数等于j =1∑2kT (j ).故i =1∑2k⎣⎡⎦⎤2ki =j =1∑2kT (j ).以2k =6为例:文案大全故a =1∑(n+1)2f (a )=k =1∑n j =1∑2kT (j )=n [T (1)+T (2)]+(n -1)[T (3)+T (4)]+…+[T (2n -1)+T (2n )]. ③由此,k =1∑162f (k )=k =1∑16(16-k )[T (2k -1)+T (2k )] ④记a=T (2k -1)+T (2k ).可得a 的取值如下表(k =1,2,…15):k =1∑162f (k )=k =1∑16(16-k )a k=783. ⑤又当k ∈{241,242,…,255}时,设k =152+r (r =16,17,…30).则k -15=152+r -15=r 152+r +15,从而r 31<r 152+r +15<r 30,于是1≤30r <1{k }<31r <2.故,⎣⎡⎦⎤1{k }=1,k ∈{241,242,…,255},又f (256)=0, 所以k =1∑240f (k )=783-15=768.呜呼!不怕繁死人,就怕繁不成!。
滑铁卢数学竞赛高中试题

滑铁卢数学竞赛高中试题一、选择题1. 已知函数\( f(x) = ax^2 + bx + c \),其中\( a, b, c \)为实数,且\( f(1) = 2 \),\( f(-1) = 0 \),\( f(2) = 6 \)。
求\( a \)的值。
2. 一个圆的半径为5,圆心位于原点,求圆上点\( P(3,4) \)到圆心的距离。
3. 若\( \sin(\alpha + \beta) = \frac{1}{2} \),\( \cos(\alpha + \beta) = \frac{\sqrt{3}}{2} \),且\( \alpha \)在第二象限,\( \beta \)在第一象限,求\( \sin(\alpha) \)的值。
二、填空题1. 计算\( \int_{0}^{1} x^2 dx \)。
2. 若\( \log_{2}8 = n \),则\( n \)的值为______。
3. 一个等差数列的前三项分别为2,5,8,求该数列的第10项。
三、解答题1. 证明:对于任意正整数\( n \),\( 1^3 + 2^3 + ... + n^3 =\frac{n^2(n+1)^2}{4} \)。
2. 一个矩形的长是宽的两倍,若矩形的周长为24,求矩形的面积。
3. 已知一个等比数列的前三项分别为3,9,27,求该数列的第5项。
四、应用题1. 一个工厂每天生产相同数量的零件,如果每天生产100个零件,工厂可以在30天内完成订单。
如果每天生产150个零件,工厂可以在20天内完成订单。
求工厂每天实际生产的零件数量。
2. 一个圆环的外圆半径是内圆半径的两倍,且圆环的面积为π。
求外圆的半径。
五、证明题1. 证明:对于任意实数\( x \),\( \cos(x) + \cos(2x) + \cos(3x) \)可以表示为一个单一的余弦函数。
六、开放性问题1. 考虑一个无限大的棋盘,每个格子可以放置一个硬币。
2005年全国高中数学联赛试题及解答

1 或1 a 5 . 3
2 2
1 7 3a 2 − 4a + 1 = ( 3a − 1)( a − 1) , 解: f ( x ) 在 ( 0, + ) 上定义,又 2a + a + 1 = 2 a + + 0; 4 8
仅当 a 1 或 a
1 () 时, 3a 2 − 4a + 1 0. 3
共 13 页
1
答案:B. 解:将正方体切去两个正三棱锥 A − ABD与 C − DBC 后 , 得 到 一个 以平 行 平 面 ABD与DBC 为上、下底面的几何体 V,V 的每个侧面都是等腰直角三角形,截面 多边形 W 的每一条边分别与 V 的底面上的一条边平行, 将 V 的侧面沿棱 AB 剪开, 展平在一张平面上,得到一个平行四边形 ABB1 A1 ,而多边形 W 的周界展开后便成 为一条与 AA1 平行的线段(如图中 E E1 ) ,显然 E E1 = AA1 ,故 l 为定值. 当 E 位于 AB 中点时,多边形 W 为正六边形,而当 E 移至 A 处时,W 为正三 角形,易知周长为定值 l 的正六边形与正三角形面积分别为 定值.
Hypatia滑铁卢数学竞赛(Grade 11)-数学Mathematics-2005-试题 exam

2005Hypatia Contest(Grade11)Wednesday,April20,20051.For numbers a and b,the notation a b means a2−4b.For example,5 3=52−4(3)=13.(a)Evaluate2 3.(b)Find all values of k such that k 2=2 k.(c)The numbers x and y are such that3 x=y and2 y=8x.Determine the values of x and y.2.Gwen and Chris are playing a game.They begin with a pile of toothpicks,and use the followingrules:•The two players alternate turns•On any turn,the player can remove1,2,3,4,or5toothpicks from the pile•The same number of toothpicks cannot be removed on two different turns•The last person who is able to play wins,regardless of whether there are any toothpicks remaining in the pileFor example,if the game begins with8toothpicks,the following moves could occur: Gwen removes1toothpick,leaving7in the pileChris removes4toothpicks,leaving3in the pileGwen removes2toothpicks,leaving1in the pileGwen is now the winner,since Chris cannot remove1toothpick.(Gwen already removed 1toothpick on one of her turns,and the third rule says that1toothpick cannot be removed on another turn.)(a)Suppose the game begins with11toothpicks.Gwen begins by removing3toothpicks.Chris follows and removes1.Then Gwen removes4toothpicks.Explain how Chris can win the game.(b)Suppose the game begins with10toothpicks.Gwen begins by removing5toothpicks.Explain why Gwen can always win,regardless of what Chris removes on his turn.(c)Suppose the game begins with9toothpicks.Gwen begins by removing2toothpicks.Explain how Gwen can always win,regardless of how Chris plays.2005Hypatia Contest Page 23.In the diagram, ABC is equilateral with side length4.Points P ,Q and R are chosen on sides AB ,BC and CA ,respectively,such that AP =BQ =CR =1.BQR CA P(a)Determine the exact area of ABC .Explain how you got your answer.(b)Determine the exact areas of P BQ and P QR .Explain how you got your answers.4.An arrangement of a set is an ordering of all of the numbers in the set,in which each number appears exactly once.For example,312and 231are two of the possible arrangements of {1,2,3}.(a)Determine the number of triples (a,b,c )where a ,b and c are three different numberschosen from {1,2,3,4,5}with a <b and b >c .Explain how you got your answer.(b)How many arrangements of {1,2,3,4,5,6}contain the digits 254consecutively in thatorder?Explain how you got your answer.(c)A local peak in an arrangement occurs where there is a sequence of 3numbers in thearrangement for which the middle number is greater than both of its neighbours.For example,the arrangement 35241of {1,2,3,4,5}contains 2local peaks.Determine,with justification,the average number of local peaks in all 40320possible arrangements of {1,2,3,4,5,6,7,8}.。
学科竞赛-NOIP2005普及组复赛试题(附题解)

NOIP2005普及组复赛试题(附题解)noip2005普及组解题报告陶陶摘苹果【文件名】: apple.pas/c/cpp【问题描述】陶陶家的院子里有一棵苹果树,每到秋天树上就会结出10个苹果。
苹果成熟的时候,陶陶就会跑去摘苹果。
陶陶有个30厘米高的板凳,当她不能直接用手摘到苹果的时候,就会踩到板凳上再试试。
现在已知10个苹果到地面的高度,以及陶陶把手伸直的时候能够达到的最大高度,请帮陶陶算一下她能够摘到的苹果的数目。
假设她碰到苹果,苹果就会掉下来。
【输入文件】输入文件apple.in包括两行数据。
第一行包含10个100到200之间(包括100和200)的整数(以厘米为单位)分别表示10个苹果到地面的高度,两个相邻的整数之间用一个空格隔开。
第二行只包括一个100到120之间(包含100和120)的整数(以厘米为单位),表示陶陶把手伸直的时候能够达到的最大高度。
【输出文件】输出文件apple.out包括一行,这一行只包含一个整数,表示陶陶能够摘到的苹果的数目。
【样例输入】100 200 150 140 129 134 167 198 200 111110【样例输出】5分析:拿到这道题,我觉得还是比较简单的。
由于数据范围都不超过200,用integer完全就足够了,具体步骤如下:1.读入2.循环1 to 103.判断,找出最优解4.输出【程序清单】program apple;vari,n,s:integer;a:array[1..10] of integer;beginassign(input,'apple.in');reset(input);for i:=1 to 10 do read(a[i]);read(n);s:=0;for i:=1 to 10 doif n+30>=a[i] then s:=s+1;close(input);assign(output,'apple.out');rewrite(output);write(s);close(output);end.校门外的树【文件名】tree.pas/c/cpp【问题描述】某校大门外长度为L的马路上有一排树,每两棵相邻的树之间的间隔都是1米。
pgg2005真题答案及解析

pgg2005真题答案及解析PGG2005是一项备受关注的考试,对于许多考生来说,获取真题答案及解析是提高考试成绩的关键。
在本文中,我们将探讨PGG2005真题的重要性,并提供一些解析技巧,帮助考生更好地应对这一考试。
首先,了解PGG2005真题的重要性是至关重要的。
通过研究往年真题,考生可以更好地了解考试的难度、命题思路以及重点考查的内容。
这有助于考生对备考进行更有针对性的规划和准备。
通过解析真题,考生能够深入了解考题背后的知识点和解题思路,从而提高解题的准确性和速度。
接下来,我们将为考生提供一些解析PGG2005真题的技巧。
首先,阅读题目时要仔细理解题意,确保对题目的要求有清晰的理解。
其次,理清思路,逐步分析解题思路,将问题拆解成更小的部分,以帮助解题。
然后,合理运用已掌握的知识和技巧进行解题,避免过度复杂化问题,保持问题的简洁性和直观性。
最后,检查答案的合理性和准确性,确保解答完整,没有遗漏。
这些技巧可以帮助考生更好地应对PGG2005真题。
在解析PGG2005真题时,考生还应注意一些常见考点和解题思路。
首先,对于阅读理解题,考生应注重理解文章的主旨和要点,从中提取重要信息,并根据问题要求进行答题。
其次,对于选择题,考生要注意选项的细微差别,并灵活运用排除法进行选择。
此外,考生还应多做一些模拟题,加强自己的解题技巧和应对能力。
通过解析PGG2005真题,考生可以更全面地了解考试的内容和要求,从而制定更高效的备考策略。
在备考过程中,考生要注重系统性的学习和复习,合理安排时间,加强对重点知识的掌握和理解。
同时,考生还应进行自我评估,找出自己的薄弱环节,并有针对性地加强练习和巩固。
最后,希望通过本文提供的PGG2005真题答案及解析,能够帮助考生更好地应对这一考试,提高自己的考试成绩。
在备考过程中,要保持积极的心态和良好的学习习惯,相信自己的努力一定会有所回报。
加油吧,考生们!祝你们取得优异的考试成绩!。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
1.(a)Answer:a=5Since(a,a)lies on the line3x−y=10,then3a−a=10or2a=10or a=5.(b)Answer:(6,2)Solution1To get from A to B,we move2units to the right and1unit up.xSince C lies on the same straight line as A and B,then to get from B to C we move2 units to the right and1unit up twice,or4units to the right and2units up.Thus,the coordinates of C are(6,2).Solution2Label the origin as O and drop a perpendicular from C to P on the x-axis.xThen AOB is similar to CP B since both are right-angled and they have equal angles at B.Since BC=2AB,then CP=2AO=2(1)=2and BP=2BO=2(2)=4.Therefore,the coordinates of C are(2+4,0+2)=(6,2).(c)By the Pythagorean Theorem,AO2=AB2−OB2=502−402=900,so AO=30.Therefore,the coordinates of A are(0,30).By the Pythagorean Theorem,CD2=CB2−BD2=502−482=196,so CD=14.x Therefore,the coordinates of C are(40+48,14)=(88,14). Since M is the midpoint of AC,then the coordinates of M are1 2(0+88),12(30+14)=(44,22)2.(a)Answer:x=−2Solution1Since y=2x+3,then4y=4(2x+3)=8x+12.Since4y=8x+12and4y=5x+6,then8x+12=5x+6or3x=−6or x=−2.Solution2Since4y=5x+6,then y=54x+64=54x+32.Since y=2x+3and y=54x+32,then2x+3=54x+32or34x=−32or x=−2.Solution3Since the second equation contains a“5x”,we multiply thefirst equation by52to obtaina5x term,and obtain52y=5x+152.Subtracting this from4y=5x+6,we obtain32y=−32or y=−1.Since y=−1,then−1=2x+3or2x=−4or x=−2.(b)Answer:a=6Solution1Adding the three equations together,we obtain a−3b+b+2b+7c−2c−5c=−10+3+13 or a=6.Solution2Multiplying the second equation by3,we obtain3b−6c=9.Adding this new equation to thefirst equation,we obtain c=−1.Substituting this back into the original second equation,we obtain b=3+2c=1.Substituting into the third equation,a=−2b+5c+13=−2−5+13=6.(c)Solution1Let J be John’s score and M be Mary’s score.Since two times John’s score was60more than Mary’s score,then2J=M+60.Since two times Mary’s score was90more than John’s score,then2M=J+90.Adding these two equations,we obtain2J+2M=M+J+150or J+M=150orJ+M2=75.Therefore,the average of their two scores was75.(Note that we didn’t have to solve for their individual scores.)Solution2Let J be John’s score and M be Mary’s score.Since two times John’s score was60more than Mary’s score,then2J=M+60,so M=2J−60.Since two times Mary’s score was90more than John’s score,then2M=J+90.Substituting thefirst equation into the second,we obtain2(2J−60)=J+904J−120=J+903J=210J=70Substituting into M=2J−60gives M=80.Therefore,the average of their scores(ie.the average of70and80)is75.3.(a)Answer:x=50Simplifying using exponent rules,2(1612)+2(816)=2((24)12)+2((23)16)=2(248)+2(248)=4(248)=22(248)=250Therefore,since2x=2(1612)+2(816)=250,then x=50.(b)Solution1We factor the given equation(f(x))2−3f(x)+2=0as(f(x)−1)(f(x)−2)=0.Therefore,f(x)=1or f(x)=2.If f(x)=1,then2x−1=1or2x=2or x=1.If f(x)=2,then2x−1=2or2x=3or x=3..Therefore,the values of x are x=1or x=32Solution2Since f(x)=2x−1and(f(x))2−3f(x)+2=0,then(2x−1)2−3(2x−1)+2=04x2−4x+1−6x+3+2=04x2−10x+6=02x2−5x+3=0(x−1)(2x−3)=0Therfore,x=1or x=3.24.(a)Answer:1415Solution1The possible pairs of numbers on the tickets are(listed as ordered pairs):(1,2),(1,3), (1,4),(1,5),(1,6),(2,3),(2,4),(2,5),(2,6),(3,4),(3,5),(3,6),(4,5),(4,6),and(5,6).There arefifteen such pairs.(We treat the pair of tickets numbered2and4as being the same as the pair numbered4and2.)The pairs for which the smaller of the two numbers is less than or equal to4are(1,2), (1,3),(1,4),(1,5),(1,6),(2,3),(2,4),(2,5),(2,6),(3,4),(3,5),(3,6),(4,5),and(4,6).There are fourteen such pairs.Therefore,the probability of selecting such a pair of tickets is14.15Solution 2We find the probability that the smaller number on the two tickets is NOT less than or equal to 4.Therefore,the smaller number on the two tickets is at least 5.Thus,the pair of numbers must be 5and 6,since two distinct numbers less than or equal to 6are being chosen.As in Solution 1,we can determine that there are fifteen possible pairs that we can se-lected.Therefore,the probability that the smaller number on the two tickets is NOT less than orequal to 4is 115,so the probability that the smaller number on the two tickets IS less than or equal to 4is 1−115=1415.(b)Solution 1Since ∠HLP =60◦and ∠BLP =30◦,then ∠HLB =∠HLP −∠BLP =30◦.Also,since ∠HLP =60◦and ∠HP L =90◦,then ∠LHP =180◦−90◦−60◦=30◦.Therefore, HBL is isosceles and BL =HB =400m.In BLP ,BL =400m and ∠BLP =30◦,so LP =BL cos(30◦)=400 √32 =200√3m.Therefore,the distance between L and P is 200√3m.Solution 2Since ∠HLP =60◦and ∠BLP =30◦,then ∠HLB =∠HLP −∠BLP =30◦.Also,since ∠HLP =60◦and ∠HP L =90◦,then ∠LHP =180◦−90◦−60◦=30◦.Also,∠LBP =60◦.Let LP =x .HSince BLP is 30◦-60◦-90◦,then BP :LP =1:√3,so BP =1√3LP =1√3x .Since HLP is 30◦-60◦-90◦,then HP :LP =√3:1,so HP =√3LP =√3x .But HP =HB +BP so√3x =400+1√3x3x =400√3+x2x =400√3x =200√3Therefore,the distance from L to P is 200√3m.5.(a)Answer:(6,5)After 2moves,the goat has travelled 1+2=3units.After 3moves,the goat has travelled 1+2+3=6units.Similarly,after n moves,the goat has travelled a total of 1+2+3+···+n units.For what value of n is 1+2+3+···+n equal to 55?The fastest way to determine the value of n is by adding the first few integers until we obtain a sum of 55.This will be n =10.(We could also do this by remembering that 1+2+3+···+n =12n (n +1)and solving for n this way.)So we must determine the coordinates of the goat after 10moves.We consider first the x -coordinate.Since starting at (0,0)the goat has moved 2units in the positive x direction,4units in the negative x direction,6units in the positive x direction,8units in the negative x direction and 10units in the positive x direction,so its x coordinate should be 2−4+6−8+10=6.Similarly,its y -coordinate should be 1−3+5−7+9=5.Therefore,after having travelled a distance of 55units,the goat is at the point (6,5).(b)Solution 1Since the sequence 4,4r ,4r 2is also arithmetic,then the difference between 4r 2and 4r equals the difference between 4r and 4,or4r 2−4r =4r −44r 2−8r +4=0r 2−2r +1=0(r −1)2=Therefore,the only value of r is r =1.Solution 2Since the sequence 4,4r ,4r 2is also arithmetic,then we can write 4r =4+d and 4r 2=4+2d for some real number d .(Here,d is the common difference in this arithmetic sequence.)Then d =4r −4and 2d =4r 2−4or d =2r 2−2.Therefore,equating the two expressions for d ,we obtain 2r 2−2=4r −4or 2r 2−4r +2=0or r 2−2r +1=0or (r −1)2=0.Therefore,the only value of r is r =1.6.(a)Answer:4πFirst,we notice that whenever an equilateral triangle of side length 3is placed inside acircle of radius3with two of its vertices on the circle,then the third vertex will be at the centre of the circle.This is because if we place XY Z with Y and Z on the circle and connect Y and Z to the centre O,then OY=OZ=3,so OY Z is equilateral(since all three sides have length3).Thus XY Z and OY Z must be the same,so X is at the same point as O. Thus,in the starting position,A is at the centre of the circle.As the triangle is rotated about C,the point B traces out an arc of a circle of radius3. What fraction of the circle is traced out?When point A reaches point A1on the circle,we have AC=3and CA1=3.Since A is at the centre of the circle,then AA1=3as well,so AA1C is equilateral,and∠A1CA=60◦, so the triangle has rotated through60◦.1Therefore,B has traced out60◦360◦=16of a circle of radius3.Notice that A has also traced out an arc of the same length.When A reaches the circle, we have A and C on the circle,so B must be at the centre of the circle.Thus,on the next rotation,B again rotates through16of a circle of radius3as it movesto the circle.On the third rotation,the triangle rotates about B,so B does not move.After three rotations,the triangle will have A at the centre and B and C on the circle,with the net result that the triangle has rotated180◦about the centre of the circle.Thus,to return to its original position,the triangle must undergo three more of these rotations,and B will behave in the same way as it did for thefirst three rotations.Thus,in total,B moves four times along an arc equal to16of a circle of radius3.Therefore,the distance travelled by B is4(16)(2π(3))=4π.(b)In order to determine CD,we must determine one of the angles(or at least some infor-mation about one of the angles)in BCD.To do this,we look at∠A use the fact that∠A+∠C=180◦.ADCB 5674Using the cosine law in ABD ,we obtain72=52+62−2(5)(6)cos(∠A )49=61−60cos(∠A )cos(∠A )=15Since cos(∠A )=15and ∠A +∠C =180◦,then cos(∠C )=−cos(180◦−∠A )=−15.(We could have calculated the actual size of ∠A using cos(∠A )=15and then used this to calculate the size of ∠C ,but we would introduce the possibility of rounding error by doing this.)Then,using the cosine law in BCD ,we obtain72=42+CD 2−2(4)(CD )cos(∠C )49=16+CD 2−8(CD ) −150=5CD 2+8CD −1650=(5CD +33)(CD −5)So CD =−335or CD =5.(We could have also determined these roots using the quadratic formula.)Since CD is a length,it must be positive,so CD =5.(We could have also proceeded by using the sine law in BCD to determine ∠BDC and then found the size of ∠DBC ,which would have allowed us to calculate CD using the sine law.However,this would again introduce the potential of rounding error.)7.(a)Answer:Maximum =5,Minimum =1We rewrite by completing the square as f (x )=sin 2x −2sin x +2=(sin x −1)2+1.Therefore,since (sin x −1)2≥0,then f (x )≥1,and in fact f (x )=1when sin x =1(which occurs for instance when x =90◦).Thus,the minimum value of f (x )is 1.To maximize f (x ),we must maximize (sin x −1)2.Since −1≤sin x ≤1,then (sin x −1)2is maximized when sin x =−1(for instance,when x =270◦).In this case,(sin x −1)2=4,so f (x )=5.Thus,the maximum value of f (x )is 5.(b)From the diagram,the x -intercepts of the parabola are x =−k and x =3k .xy Since we are given that y =−14(x −r )(x −s ),then the x -intercepts are r and s ,so r and s equal −k and 3k in some order.Therefore,we can rewrite the parabola as y =−14(x −(−k ))(x −3k ).Since the point (0,3k )lies on the parabola,then 3k =−14(0+k )(0−3k )or 12k =3k 2or k 2−4k =0or k (k −4)=0.Thus,k =0or k =4.Since the two roots are distinct,then we cannot have k =0(otherwise both x -intercepts would be 0).Thus,k =4.This tells us that the equation of the parabola is y =−14(x +4)(x −12)or y =−14x 2+2x +12.We still have to determine the coordinates of the vertex,V .Since the x -intercepts of the parabola are −4and 12,then the x -coordinate of the vertex is the average of these intercepts,or 4.(We could have also used the fact that the x -coordinate is −b2a =−22(−14).)Therefore,the y -coordinate of the vertex is y =−14(42)+2(4)+12=16.Thus,the coordinates of the vertex are (4,16).8.(a)We look at the three pieces separately.If x <−4,f (x )=4so g (x )= 25−[f (x )]2=√25−42=√9=3.So g (x )is the horizontal line y =3when x <−4.If x >5,f (x )=−5so g (x )= 25−[f (x )]2= 25−(−5)2=√0=0.So g (x )is the horizontal line y =0when x >5.So far,our graph looks like this:If−4≤x≤5,f(x)=−x so g(x)=25−[f(x)]2=25−(−x)2=√25−x2.What is this shape?If y=g(x),then we have y=√25−x2or y2=25−x2or x2+y2=25.Therefore,this shape is a section of the upper half(since y is a positive square-root)of the circle x2+y2=25,ie.the circle with centre(0,0)and radius5.We must check the endpoints. When x=−4,we have g(−4)=25−(−4))2=3.When x=5,we have g(5)=√25−52=0.Therefore,the section of the circle connects up with the other two sections of our graph already in place.Thus,ourfinal graph is:(b)Solution 1Let the centres of the two circles be O 1and O 2.Join A and B to O 1and B and C to O 2.Designate two points W and X on either side of A on one tangent line,and two points Y and Z oneither side of C on the other tangent line.ZLet ∠XAB =θ.Since W X is tangent to the circle with centre O 1at A ,then O 1A is perpendicular to W X ,so ∠O 1AB =90◦−θ.Since O 1A =O 1B because both are radii,then AO 1B is isosceles,so ∠O 1BA =∠O 1AB =90◦−θ.Since the two circles are tangent at B ,then the line segment joining O 1and O 2passes through B ,ie.O 1BO 2is a straight line segment.Thus,∠O 2BC =∠O 1BA =90◦−θ,by opposite angles.Since O 2B =O 2C ,then similarly to above,∠O 2CB =∠O 2BC =90◦−θ.Since Y Z is tangent to the circle with centre O 2at C ,then O 2C is perpendicular to Y Z .Thus,∠Y CB =90◦−∠O 2CB =θ.Since ∠XAB =∠Y CB ,then W X is parallel to Y Z ,by alternate angles,as required.Solution2Let the centres of the two circles be O1and O2.Join A and B to O1and B and C to O2.Since AO1and BO1are radii of the same circle,AO1=BO1so AO1B is isosceles,so ∠O1AB=∠O1BA.Since BO2and CO2are radii of the same circle,BO2=CO2so BO2C is isosceles,so ∠O2BC=∠O2CB.Since the two circles are tangent at B,then O1BO2is a line segment(ie.the line segment joining O1and O2passes through the point of tangency of the two circles).Since O1BO2is straight,then∠O1BA=∠O2BC,by opposite angles.Thus,∠O1AB=∠O1BA=∠O2BC=∠O2CB.This tells us that AO1B is similar to BO2C,so∠AO1B=∠BO2C or∠AO1O2=∠CO2O1.Therefore,AO1is parallel to CO2,by alternate angles.But A and C are points of tangency,AO1is perpendicular to the tangent line at A and CO2is perpendicular to the tangent line at C.Since AO1and CO2are parallel,then the two tangent lines must be parallel.9.(a)Solution1We have(x−p)2+y2=r2and x2+(y−p)2=r2,so at the points of intersection,(x−p)2+y2=x2+(y−p)2x2−2px+p2+y2=x2+y2−2py+p2−2px=−2pyand so x=y(since we may assume that p=0otherwise the two circles would coincide).Therefore,a and b are the two solutions of the equation(x−p)2+x2=r2or2x2−2px+(p2−r2)=0or x2−px+12(p2−r2)=0.Using the relationship between the sum and product of roots of a quadratic equation andits coefficients,we obtain that a+b=p and ab=12(p2−r2).(We could have solved for a and b using the quadratic formula and calculated these di-rectly.)So we know that a+b=p.Lastly,a2+b2=(a+b)2−2ab=p2−2 12(p2−r2)=r2,as required.Solution2Since the circles are reflections of one another in the line y=x,then the two points of intersection must both lie on the line y=x,ie.A has coordinates(a,a)and B has coordinates(b,b).Therefore,(a−p)2+a2=r2and(b−p)2+b2=r2,since these points lie on both circles.Subtracting the two equations,we get(b −p )2−(a −p )2+b 2−a 2=0((b −p )−(a −p ))((b −p )+(a −p ))+(b −a )(b +a )=0(b −a )(a +b −2p )+(b −a )(b +a )=0(b −a )(a +b −2p +b +a )=02(b −a )(a +b −p )=0Since a =b ,then we must have a +b =p ,as required.Since a +b =p ,then a −p =−b ,so substituting back into (a −p )2+a 2=r 2gives (−b )2+a 2=r 2,or a 2+b 2=r 2,as required.(b)We first draw a diagram.yxWe know that C has coordinates (p,0)and D has coordinates (0,p ).Thus,the slope of line segment CD is −1.Since the points A and B both lie on the line y =x ,then the slope of line segment AB is 1.Therefore,AB is perpendicular to CD ,so CADB is a kite,and so its area is equal to 12(AB )(CD ).(We could derive this by breaking quadrilateral CADB into CAB and DAB .)Since C has coordinates (p,0)and D has coordinates (0,p ),then CD = p 2+(−p )2= 2p 2.(We do not know if p is positive,so this is not necessarily equal to √2p .)We know that A has coordinates (a,a )and B has coordinates (b,b ),soAB = (a −b )2+(a −b )2=√2a 2−4ab +2b 2= 2(a 2+b 2)−4ab = 2r 2−4 12(p 2−r 2) = 4r 2−2p 2Therefore,the area of quadrilateral CADB is 12(AB )(CD )=124r 2−2p 2 2p 2= 2r 2p 2−p 4To maximize this area,we must maximize 2r 2p 2−p 4=2r 2(p 2)−(p 2)2.Since r is fixed,we can consider this as a quadratic polynomial in p 2.Since the coefficient of (p 2)2is negative,then this is a parabola opening downwards,so we find its maximum value by finding its vertex.The vertex of 2r 2(p 2)−(p 2)2is at p 2=−2r 22(−1)=r 2.So the maximum area of the quadrilateral occurs when p is chosen so that p 2=r 2.Since p 2=r 2,then (a +b )2=p 2=r 2so a 2+2ab +b 2=r 2.Since a 2+b 2=r 2,then 2ab =0so either a =0or b =0,and so either A has coordinates (0,0)or B has coordinates (0,0),ie.either A is the origin or B is the origin.(c)In (b),we calculated that AB = 4r 2−2p 2=√2 2r 2−p 2.Since r and p are integers (and we assume that neither r nor p is 0),then 2r 2−p 2=0,so the minimum possible non-negative value for 2r 2−p 2is 1,since 2r 2−p 2must be an integer.Therefore,the minimum possible distance between A and B should be √2√1=√2.Can we find positive integers p and r that give us this value?Yes –if r =5and p =7,then 2r 2−p 2=1,so AB =√2.(There are in fact an infinite number of positive integer solutions to the equation 2r 2−p 2=1or equivalently p 2−2r 2=−1.This type of equation is called Pell’s Equation.)10.(a)We proceed directly.On the first pass from left to right,Josephine closes all of the even numbered lockers,leaving the odd ones open.The second pass proceeds from right to left.Before the pass,the lockers which are open are 1,3,...,47,49.On the second pass,she shuts lockers 47,43,39, (3)The third pass proceeds from left to right.Before the pass,the lockers which are open are 1,5,...,45,49.On the third pass,she shuts lockers 5,13, (45)This leaves lockers 1,9,17,25,33,41,49open.On the fourth pass,from right to left,lockers 41,25and 9are shut,leaving 1,17,33,49.On the fifth pass,from left to right,lockers 17and 49are shut,leaving 1and 33open.On the sixth pass,from right to left,locker 1is shut,leaving 33open.Thus,f (50)=33.(b)&(c)Solution 1First,we note that if n =2k is even,then f (n )=f (2k )=f (2k −1)=f (n −1).See Solution 2for this justification.Therefore,we only need to look for odd values of n in parts (b)and (c).Suppose that there was an n so that f (n )=2005,ie.2005is the last locker left open.On the first pass,Josephine closes every other locker starting at the beginning,so she closes all lockers numbered m with m ≡0(mod 2).This leaves only odd-numbered lockers open,ie.only lockers m with m ≡1or 3(mod 4).On her second pass,she closes every other open locker,starting from the right-hand end.Thus,she will close every fourth locker from the original row.Since we want 2005to be left open and 2005≡1(mod 4),then she must close all lockers numbered m with m ≡3(mod 4).This leaves open only the lockers m with m ≡1(mod 4),or equivalently lockers with m ≡1or 5(mod 8).On her third pass,she closes every other open locker,starting from the left-hand end.Thus,she will close every eighth locker from the original row.Since locker1is still open,then she starts by closing locker5,and so closes all lockers mwith m≡5(mod8).But since2005≡5(mod8),then she closes locker2005on this pass,a contradiction.Therefore,there can be no integer n with f(n)=2005.Next,we show that there are infinitely many positive integers n such that f(n)=f(2005).To do this,wefirst make a table of what happens when there are2005lockers in the row.We record the pass#,the direction of the pass,the leftmost locker that is open,therightmost locker that is open,all open lockers before the pass,which lockers will be closedon the pass,and which lockers will be left open after the pass:Pass#Dir.L Open R Open Open To close Leaves Open 1L to R12005All≡0(mod2)≡1(mod2) 2R to L12005≡1,3(mod4)≡3(mod4)≡1(mod4) 3L to R12005≡1,5(mod8)≡5(mod8)≡1(mod8) 4R to L12001≡1,9(mod16)≡9(mod16)≡1(mod16) 5L to R12001≡1,17(mod32)≡17(mod32)≡1(mod32) 6R to L11985≡1,33(mod64)≡33(mod64)≡1(mod64) 7L to R11985≡1,65(mod128)≡65(mod128)≡1(mod128) 8R to L11921≡1,129(mod256)≡1(mod256)≡129(mod256) 9L to R1291921≡129,385(mod512)≡385(mod512)≡129(mod512) 10R to L1291665≡129,641(mod1024)≡129(mod1024)≡641(mod1024) 11L to R6411665≡641,1665(mod2048)≡1665(mod2048)≡641(mod2048) Since there is only one integer between1and2005congruent to641(mod2048),thenthere is only one locker left open:locker641.Notice also that on any pass s,the“class”of lockers which are closed depends on what thenumber of the leftmost(on an odd-numbered pass)or rightmost(on an even-numberedpass)open locker number is congruent to mod2s.Consider n=2005+22a,where22a>2005,ie.a≥6.We show that f(n)=f(2005)=641.(See Solution2for a justification of why we mighttry these values of n.)Suppose we were to try to make a table as above to calculate f(n).Then thefirst11passes in the table would be identical to the table above,except for therightmost open number;this number in the new table would be the number above plus22a.What will happen after pass11?After pass11,the lockers which are open are lockers with numbers≡641(mod2048).Thus,the leftmost open locker is641and the rightmost is22a+641.As the12th pass starts,the lockers which are still open are those with numbers≡641or2689(mod212).Since the rightmost open locker number(22a+641)is congruent to641(mod212),then alllockers with numbers≡2689(mod212)are closed,leaving open only those lockers withnumbers≡641(mod212).So after this12th pass,the lockers which are open are641,641+212,641+2(212),641+3(212),...,641+22a−12(212)=641+22a.The number of open lockers is22a−12+1.If we can now show that whenever we start with a number of lockers of the form22c+1,thelast locker remaining open is the leftmost locker,then we will be done,since of the lockersleft open above(22a−12+1of them,ie.2to an even power plus1),then the last locker re-maining open will be the leftmost one,that is locker641,so f(22a+2005)=641=f(2005).So consider a row of22c+1lockers.Notice that on any pass,if the number of lockers is odd,then the number of lockers whichwill be closed is one-half of one less than the total number of lockers,and thefirst andlast lockers will be left open.So on thefirst pass,there are22c−1lockers closed,leaving22c+1−22c−1=22c−1+1lockersopen,ie.an odd number of lockers open.On the next pass,there are22c−2lockers closed(since there are an odd number of lockersopen to begin),leaving22c−2+1lockers open.This continues,until there are21+1=3lockers open just before an even-numbered(ie.right to left)pass.Thus,the middle of these three lockers will be closed,leaving only theoriginal leftmost and rightmost lockers open.On the last pass(an odd-numbered pass from left to right),the rightmost locker will beclosed,leaving only the leftmost locker open.Therefore,starting with a row of22c+1open lockers,the leftmost locker will be the lastremaining open.Translating this to the above,we see that the leftmost locker of the22a−12+1still openis the last left open,ie.f(22a+2005)=641=f(2005)if a≥6.Therefore,there are infinitely many positive integers n for which f(n)=f (2005).Solution2First,we calculate f(n)for n from1to32,to get a feeling for what happens.We obtain 1,1,3,3,1,1,3,3,9,9,11,11,9,9,11,11,1,1,3,3,1,1,3,3,9,9,11,11,9,9,11,11. This will help us to establish some patterns.Next,we establish two recursive formulas for f(n).First,from our pattern,it looks like f(2m)=f(2m−1).Why is this true in general?Consider a row of2m lockers.On thefirst pass through,Josephine shuts all of the even numbered lockers,leaving open lockers1,3,...,2m−1.These are exactly the same open lockers as if she had started with2m−1lockers in total. Thus,as she starts her second pass from right to left,the process will be the same now whether she started with2m lockers or2m−1lockers.Therefore,f(2m)=f(2m−1).This tells us that we need only focus on the values of f(n)where n is odd.Secondly,we show that f(2m−1)=2m+1−2f(m).(It is helpful to connect n=2m−1to a smaller case.)Why is this formula true?Starting with2m−1lockers,the lockers left open after thefirst pass are1,3,...,2m−1, ie.m lockers in total.Suppose f(m)=p.As Josephine begins her second pass,which is from right to left,we can think of this as being like thefirst pass through a row of m lockers.Thus,the last open locker will be the p th locker,counting from the right hand end,from the list1,3,...,2m−1.Thefirst locker from the right is2m−1=2m+1−2(1),the second is2m−3=2m+1−2(2), and so on,so the p th locker is2m+1−2p.Therefore,thefinal open locker is2m+1−2p,ie.f(2m−1)=2m+1−2p=2m+1−2f(m). Using these two formulae repeatedly,f(4k+1)=f(2(2k+1)−1)=2(2k+1)+1−2f(2k+1)=4k+3−2f(2(k+1)−1)=4k+3−2(2(k+1)+1−2f(k+1))=4k+3−2(2k+3−2f(k+1))=4f(k+1)−3andf(4k+3)=f(2(2k+2)−1)=2(2k+2)+1−2f(2k+2)=4k+5−2f(2k+1)=4k+5−2f(2(k+1)−1)=4k+5−2(2(k+1)+1−2f(k+1))=4k+5−2(2k+3−2f(k+1))=4f(k+1)−1From our initial list of values of f(n),it appears as if f(n)cannot leave a remainder of5 or7when divided by8.So we use these recursive relations once more to try to establish this:f(8l+1)=4f(2l+1)−3(since8l+1=4(2l)+1)=4(2l+3−2f(l+1))−3=8l+9−8f(l+1)=8(l−f(l+1))+9f(8l+3)=4f(2l+1)−1(since8l+3=4(2l)+3)=4(2l+3−2f(l+1))−1=8l+11−8f(l+1)=8(l−f(l+1))+11Similarly,f(8l+5)=8l+9−8f(l+1)and f(8l+7)=8l+11−8f(l+1). Therefore,since any odd positive integer n can be written as8l+1,8l+3,8l+5or8l+7, then for any odd positive integer n,f(n)is either9more or11more than a multiple of8. Therefore,for any odd positive integer n,f(n)cannot be2005,since2005is not9more or11more than a multiple of8.Thus,for every positive integer n,f(n)=2005,since we only need to consider odd values of n.Next,we show that there are infinitely many positive integers n such that f(n)=f(2005). We do this by looking at the pattern we initially created and conjecturing thatf(2005)=f(2005+22a)if22a>2005.(We might guess this by looking at the connection between f(1)and f(3) with f(5)and f(7)and then f(1)through f(15)with f(17)through f(31).In fact,it appears to be true that f(m+22a)=f(m)if22a>m.)Using our formulae from above,f(2005+22a)=4f(502+22a−2)−3(2005+22a=4(501+22a−2)+1) =4f(501+22a−2)−3=4(4f(126+22a−4)−3)−3(501+22a−2=4(125+22a−4)+1)=16f(126+22a−4)−15=16f(125+22a−4)−15=16(4f(32+22a−6)−3)−15(125+22a−4=4(31+22a−6)+1)=64f(32+22a−6)−63=64f(31+22a−6)−63=64(4f(8+22a−8)−1)−63(31+22a−6=4(7+22a−8)+3)=256f(8+22a−8)−127=256f(7+22a−8)−127=256(4f(2+22a−10)−1)−127(7+22a−8=4(1+22a−10)+3)=1024f(2+22a−10)−383=1024f(1+22a−10)−383(Notice that we could have removed the powers of2from inside the functions and used this same approach to show that f(2005)=1024f(1)−383=641.)But,f(22b+1)=1for every positive integer b.Why is this true?We can prove this quickly by induction.For b=1,we know f(5)=1.Assume that the result is true for b=B−1,for some positive integer B≥2.Then f(22B+1)=f(4(22B−2)+1)=4f(22B−2+1)−3=4(1)−3=1by our induction hypothesis.Therefore,if a≥6,then f(1+22a−10)=f(1+22(a−5))=1sof(2005+22a)=1024(1)−383=641=f(2005)so there are infinitely many integers n for which f(n)=f(2005).。
