2019年数学竞赛试题及答案

2019年全国高中数学联赛试题及解答

全国高中数学联合竞赛试题（A 卷）一试一、填空题（本大题共8小题，每小题8分，共64分）1. 若正数,a b 满足()2362log 3log log a b a b +=+=+，则11a b+的值为________．答案：设连等式值为k ，则232,3,6k k ka b a b --==+=，可得答案108分析：对数式恒等变形问题，集训队讲义专门训练并重点强调过2. 设集合3|12b a b a ⎧⎫+≤≤≤⎨⎬⎩⎭中的最大元素与最小你别为,M m ，则M m -的值为______．答案：33251b a +≤+=，33b a a a+≥+≥，均能取到，故答案为5-分析：简单最值问题，与均值、对勾函数、放缩有关，集训队讲义上有类似题 3. 若函数()21f x x a x =+-在[0,)+∞上单调递增，则实数a 的取值范围是______．答案：零点分类讨论去绝对值，答案[]2,0-分析：含绝对值的函数单调性问题，集训队讲义专门训练并重点强调过4. 数列{}n a 满足12a =，()()*1221n n n a a n N n ++=∈+，则2014122013a a a a =+++______．答案：()1221n n n aa n ++=+，迭乘得()121n n a n -=+，()212232421n n S n -=+⨯+⨯+++，乘以公比错位相减，得2n n S n =，故答案为20152013．分析：迭乘法求通项，等差等比乘积求前n 项和，集训队讲义专门训练并重点强调过5. 正四棱锥P ABCD -中，侧面是边长为1的正三角形，,M N 分别是边,AB BC 的中点，则异面直线MN与PC 之间的距离是________．答案：OB 为公垂线方向向量，故距离为12OB =分析：异面直线距离，也可以用向量法做，集训队讲义专门练并重点强调过6. 设椭圆Γ的两个焦点是12,F F ，过点1F 的直线与Γ交于点,P Q ．若212PF F F =，且1134PF QF =，则椭圆Γ的短轴与长轴的比值为________．答案：不妨设焦点在x 轴（画图方便），设114,3PF QF ==，焦距为2c ，224a c =+，可得△2PQF 三边长为7,21,2c c +，过2F 作高，利用勾股可得5c =．分析：椭圆中常规计算，与勾股定理、解三角形、斯特瓦尔特等有关，集训队讲义训练过相关7. 设等边三角形ABC 的内切圆半径为2，圆心为I ．若点P 满足1PI =，则△APB 与△APC 的面积之比的最大值为________．答案：sin sin APB APC S PABS PAC ∠=∠，又两角和为60最大，即AP 与(),1I 切于对称轴右侧2分析：平面几何最值、面积、三角函数、轨迹8. 设,,,A B C D 是空间中四个不共面的点，以12的概率在每对点之间连一条边，任意两点之间是否连边是相互独立的，则,A B 之间可以用空间折线（一条边或者若干条边组成）连结的概率为_______．答案：总连法64种，按由A 到B 最短路线的长度分类．长度为1，即AB 连其余随意，32种；长度为2，即AB 不连，ACB 或ADB 连，其余随意，ACB 连8种，故共88214+-=种（一定注意,ACB ADB 同时连被算了2次，根据CD 是否连有2种情形）；长度为3，两种情形考虑ACDB ，ACDB 连、,,AB CB AD 均不连只有1种，故连法为2种；综上，答案483644=分析：组合计数，分类枚举，难度不大但容易算错，集训队讲义训练过类似题目二、解答题（本大题共3小题，共56分）9. （本题满分16分）平面直角坐标系xOy 中，P 是不在x 轴上的一个动点，满足条件：过P 可作抛物线24y x =的两条切线，两切点连线P l 与PO 垂直．设直线P l 与直线PO ，x 轴的交点分别为,Q R ．（1）证明：R 是一个定点；（2）求PQQR的最小值．答案：（1）设(),P a b ，()()1122,,,A x y B x y ，0,0a b ≠≠，()11:2PA yy x x =+，()22:2PB yy x x =+ 故,A B 两点均适合方程()2by a x =+，利用垂直，可得2a =-，故交点为定点()2,0（2）∵2a =-，故,2PO PR b bk k =-=-，设OPR α∠=，则α为锐角，1tan PQ QR α=，利用两角差的正切公式，可得282PQ b QR b+=≥．分析：涉及圆锥曲线切点弦方程、两直线夹角公式、不等式求最值，集训队讲义专门训练并重点过10. （本题满分20分）数列{}n a 满足16a π=，()()*1arctan sec n n a a n N +=∈．求正整数m ，使得121sin sin sin 100m a a a ⋅⋅⋅=．答案：由反函数值域，知,22n a ππ⎛⎫∈- ⎪⎝⎭，2222132tan sec tan 1tan 3n n n n a a a +-==+==，1212112122311tan tan tan tan tan tan tan sin sin sin sec sec sec tan tan tan tan m m m m m m a a a a a a a a a a a a a a a a a ++⋅⋅⋅=⋅=⋅==故3333m =分析：涉及简单反三角函数、数列通项公式求法，集训队讲义对类似题目进行过训练11. （本题满分20分）确定所有的复数α，使得对任意复数()121212,,1,z z z z z z <≠，均有()()221122z z z z αααα++≠++．答案：转换命题为计算存在12,z z 使得相等时的充要条件存在12,z z 使得相等，记()()2f z z z αα=++，()()()()()1212121220f z f z z z z z z z αα-=++-+-=，则()()()1212122z z z z z z αα-=-++-，故12122222z z z z a ααα=++≥-->-，故2α<；若2α<，令12,22z i z i ααββ=-+=--，其中012αβ<<-，则12z z ≠，122i ααββ-±≤-+<，计算121212,2,2z z z z i z z i αββ+=--=-=-并代入，知()()12f z f z =．综上，满足条件的α为,2Z αα∈≥二试一、（本题满分40分）设实数,,a b c满足1a b c++=，0abc>．求证：14ab bc ca++<．a b c≥≥>，则1a≥1c≤．)ab bc ca c++-+⎭12c-，故有()()111122c c cc cc c⎛---≤-+-⎭⎝⎭由于1110,3333c-≥+≥>310c->，故原不等式成立．方法2：不妨设0a b c≥≥>，则13a≥c，设()()1f b ab bc ca ab c c=++=+-，()f b递增f⇔，()())()1f b ab a b a b⎛'=--=-⎝，()010f b'≥⇔≥⇔≤≥故()f b a；题目转化为21ac+=，a c≥，记()()222212g a a ac a a a=+-=+--()()262621g a a a⎫'=-+=-⎪⎭，由于13a≥1=，得1532a=，115,332a⎛⎫∈ ⎪⎝⎭时g'151,322⎫⎪⎝⎭时()g a在13或12max1124g g⎛⎫==⎪⎝⎭分析：一道偏函数化的不等式题，可以将其放缩为一元函数，也可以拿导数与调整法很快做出来，集训队讲义上两种方法都训练过．二、（本题满分40分）在锐角三角形ABC中，60BAC∠≠，过点,B C分别作三角形ABC的外接圆的切线,BD CE，且满足BD CE BC==．直线DE与,AB AC的延长线分别交于点,F G．设CF与BD交于点M，CE与BG交于点N．证明：AM AN=．答案：设△ABC三边为,,a b c，则BD CE a==，先计算AM，∵,BFD ABC BDF DBC BAC∠=∠∠=∠=∠，∴△BFD∽△CBA．由比例可知acDFb=，故BM BC bBDDF c==，故abBMb c=+，故由余弦定理知()2222cosab abAM c c A Bb c b c⎛⎫=+-⋅+⎪++⎝⎭222cosab abcc Cb c b c⎛⎫=++⎪++⎝⎭，整理可得此式关于,b c对称故可知22AM AN=分析：由于一旦,,a b c三边确定则图形固定，所以通过相似、比例、余弦定理计算的思路比较显然GF ED三、（本题满分50分）设{}1,2,3,,100S =．求最大的整数k ，使得S 有k 个互不相同的非空子集，具有性质：对这k 个子集中任意两个不同子集，若它们的交非空，则它们交集中的最小元素与这两个子集中的最大元素均不相同．答案：一方面，取包含1的、至少含2个元素的所有子集，共9921-个，显然满足题意；另外归纳证对于{}1,2,3,,S n =，任取()123n n -≥个子集，均存在两个的交集中最小的等于某个中最大的当3n =时，将7个非空子集分为三类：{}{}{}31,32,3，{}{}21,2，{}{}11,2,3．任取四个必有两个同类．假设n k =时命题成立，当1n k =+时，如果取出的2k 个子集中至少有12k -个不含1k +，利用归纳假设知成立；如果不含1k +的不足12k -，则至少有121k -+个含有1k +，而S 含有1k +的子集共2k 个，可以配成12k - 对，使得每对中除了公共元素1k +外，其余恰为1到n 的互补子集，这样，如果选出121k -+个，则必有两个除1k +外不交，故命题成立．综上，k 的最大值为9921-．分析：集合中的组合最值问题，比较常规的一道题，类似感觉的题集训队讲义在组合中的归纳法中有过四、（本题满分50分）设整数122014,,,x x x 模2014互不同余，整数122014,,,y y y 模2014也互不同余．证明：可将122014,,,y y y 重新排列为122014,,,z z z ，使得112220142014,,,x z x z x z +++模4028互不同余．答案：不妨设()mod 2014i i x y i ≡≡，1,2,,2014i =．下面对i y 序列进行1007次调整从而构成i z 序列：若i i x y +与10071007i i x y +++模4028不同余，则1007,i i y y +不调整；否则，交换1007,i i y y +位置，1,2,,2014i =．下证，进行1007次调整后，得到的i z 序列一定满足条件．任意挑选一列()1,2,,1007i i x z i +=，只需证其与10071007i i x z +++、()1,2,,1007,j j x z j j i +=≠、10071007j j x z +++模4028不同余即可由i z 构造方法，i i x z +与10071007i i x z +++不同余是显然的，因为不可能调整前后均同余，故只需看另两个；首先，对于不同的,i j ，2i 与2j 模4028不同余，否则会导致()mod 2014i j ≡．若,i j y y 均未调整，则()2mod 2014i i x z i +≡，()100710072mod 2014j j j j x z x z j +++≡+≡，故成立；若,i j y y 均已调整，则()21007mod 2014i i x z i +≡+，()1007100721007mod 2014j j j j x z x z j +++≡+≡+，故成立；若只有一个被调整过，不妨设i y 未调整、j y 已调整，则()2mod 2014i i x z i +≡， ()1007100721007mod 2014j j j j x z x z j +++≡+≡+，若()4028|21007i j --，则()1007|i j -，矛盾，故同样成立．综上，构造的i z 序列满足条件．全国高中数学联赛试题及解答2014高中联赛试题分析从试题类型来看，今年代数、几何、数论、组合4部分所占的比例为：代数37.3%，几何26.7%，数论16.7%，组合19.3%．这方面和历年情况差不多，但具体的知识点差别极大．一试第7题填空题可谓出人意表，虽然解答是用三角函数的方法处理的，对比历年试题，这题毫无疑问也是顶替了三角函数的位置．但本题却是一道彻头彻尾的平面几何题．从图中不难看出，最值情况在相切时取到，剩下的只是利用三角函数处理了一下计算上的问题．其余填空题中，第1~6题和往年出题风格类似，第8题概率计算略显突兀，本题几乎不需要用到计数的技巧，而是用单纯枚举的方法即可解决．放在填空题最后一题的位置不免显得难度不够．一试三道解答题中，第9题和第10题均不太难，所考知识点也和往年类似，无需多说．第11题又再次爆了冷门，考了一道复数问题．联赛已经多年没有考复数的大题了，许多学生都没有准备．可以说，这次一下戳中了学生的罩门．相信本题最终的得分率不容乐观．而本次试题中最特殊的要数加试中的平面几何题了．一反从1997年开始保持到如今的惯例，没有将平面几何题放在加试的第一题．而且本题实则为《中等数学》2012年第12期中的数学奥利匹克高中训练题中的原题，这无疑又让此题失色不少．今年的加试第一题放了一道不等式问题，虽然近几年不等式考察得较少，但是不等式一直是数学竞赛中的热门，在历年联赛中多有出现．考虑到本题难度并不大，放在联赛加试第一题还是非常合适的．加试第三题组合最值问题的出题风格一如既往，可以从很极端的情况下猜出答案，再进行证明．值得全国高中数学联赛试题及解答一提的是本题题干描述有歧义，最后一句“则它们交集中的最小元素与这两个子集中的最大元素均不相同”中，记最小元素为a ，两个最大元素为b 和c ．本句话中到底是指a 、b 、c 这3个数互不相同还是指a b ≠且a c ≠，无疑是容易让人误解的．希望今后联赛试题中能避免出现这种情况．加试第四题虽说考察的是数论中的同余知识，但更多考察的是构造法技巧，这也符合联赛加试中试题综合各方面知识的出题思想．从难度上来说本题难度不算太大，只要能从较小的数开始构造并寻找规律，找出2014的构造并不显得困难．但本题的出题背景无疑和以下题目相关：“n 为给定正整数，()122,,,n x x x 和()122,,,n y y y 均为1~2n 的一个排列，则112222,,,n n x y x y x y +++这2n 个数不可能模2n 互不同余．” 总的说来，本次联赛考察的知识点和往年比差别较大，但从试卷难度来说，和前两年是相当的．预计今年联赛的分数线可能比去年略低．。

【竞赛试题】2019年全国和高中数学联赛试卷及答案

æ 4ö 【竞赛试题】2019 年全高中数学联合竞赛一试（B 卷）参考答案及评分标准1. 评阅试卷时，请依据本评分标准. 填空题只设 8 分和 0 分两档；其他各题的评阅，请严格按照本评分标准的评分档次给分，不得增加其他中间档次.2. 如果考生的解答方法和本解答不同，只要思路合理、步骤正确，在评卷时可参考本评分标准适当划分档次评分，解答题中第 9 小题 4 分为一个档次，第 10、 11 小题 5 分为一个档次，不得增加其他中间档次．一、填空题：本大题共 8 小题，每小题 8 分，满分 64 分．1. 已知实数集合{1, 2, 3, x } 的最大元素等于该集合的所有元素之和，则 x 的值为．答案：-3 ．解：条件等价于1, 2, 3, x 中除最大数以外的另三个数之和为 0 ．显然 x < 0 ，从而1 + 2 + x = 0 ，得 x = -3 ．2. 若平面向量 a = (2m , -1) 与 b = (2m -1, 2m +1) 垂直，其中 m 为实数，则 a 的模为．答案： 10 ．解：令 2m = t ，则 t > 0 ．条件等价于 t ⋅ (t -1) + (-1) ⋅ 2t = 0 ，解得 t = 3 ．因此 a 的模为 32 + (-1)2 = 10 ．3. 设a , b Î (0, p ) ，cos a , cos b 是方程5x 2 -3x -1 = 0 的两根，则sin a sin b 的值为．答案：7 ．5解：由条件知 cos a + cos b = 3 , cos a cos b = - 1，从而5 5(s i n a sin b )2 = (1- c os 2 a )(1- c os 2 b ) = 1- cos 2 a - cos 2 b + cos 2 a cos 2 b2 2= (1+ cos a cos b )2 - (cos a + cos b )2 = ÷ æ 3ö - = 7 ． ç ÷ ç ÷ çè 5 ø çè5ø 25又由a , b Î (0, p ) 知sin a sin b > 0 ，从而sin a sin b = 7．54. 设三棱锥 P - ABC 满足 PA = PB = 3, AB = BC = CA = 2 ，则该三棱锥的体积的最大值为．答案： 2 6 ．3解：设三棱锥 P - ABC 的高为 h ．取M 为棱 AB 的中点，则h £ PM = 32 -12 = 2 2 ．当平面 PAB 垂直于平面 ABC 时， h 取到最大值 2 2 ．此时三棱锥 P - ABC 的体r n -rnn积取到最大值 1S⋅= 1 ⋅ = 2 6 ．3 D ABC3 35. 将 5 个数 2, 0, 1, 9, 2019 按任意次序排成一行，拼成一个 8 位数（首位不为 0），则产生的不同的 8 位数的个数为．答案：95 ．解：易知 2, 0, 1, 9, 2019 的所有不以 0 为开头的排列共有 4´ 4! = 96 个．其中，除了 (2, 0, 1, 9, 2019) 和 (2019, 2, 0, 1, 9) 这两种排列对应同一个数 20192019 ，其余的数互不相等．因此满足条件的 8 位数的个数为96 -1 = 95 ．6. 设整数 n > 4 ，( x + 2 的值为．答案：51． y -1)n 的展开式中x n -4 与 xy 两项的系数相等，则 nn解：注意到 ( x + 2 y -1)n= år =0C n x (2 y -1)r ．其中 x n -4 项仅出现在求和指标 r = 4 时的展开式 C 4 x n -4 (2 y -1)4中，其 x n -4 项系数为 (-1)4 C 4 = n (n -1)(n - 2)(n -3) ．n24而 xy 项仅出现在求和指标 r = n -1 时的展开式 C n -1x ⋅ (2y -1)n -1 中，其 xy 项系数为 n -1 2 n -3 n -3C n C n -1 4⋅ (-1) = (-1) 2n (n -1)(n - 2) ．因此有 n (n -1)(n - 2)(n - 3)= (-1)n -3 2n (n -1)(n - 2) ．注意到 n > 4 ，化简得24n - 3 = (-1)n -3 48 ，故只能是 n 为奇数且 n - 3 = 48 ．解得 n = 51 ．7. 在平面直角坐标系中，若以 (r +1, 0) 为圆心、 r 为半径的圆上存在一点 (a , b ) 满足b 2 ³ 4a ，则 r 的最小值为．答案： 4 ．解：由条件知 (a - r -1)2 + b 2 = r 2 ，故4a £ b 2 = r 2 - (a - r -1)2 = 2r (a -1) - (a -1)2 ．即 a 2 - 2(r -1)a + 2r +1 £ 0 ．上述关于 a 的一元二次不等式有解，故判别式(2(r -1))2 - 4(2r +1) = 4r (r - 4) ³ 0 ，解得 r ³ 4 ．经检验，当 r = 4 时， (a , b ) = (3, 2 3) 满足条件．因此 r 的最小值为 4 ．8. 设等差数列{a n } 的各项均为整数，首项 a 1 = 2019 ，且对任意正整数 n ，总存在正整数 m ，使得 a 1+ a 2 ++ a n = a m ．这样的数列{a n } 的个数为．答案：5 ．解：设{a n } 的公差为 d ．由条件知 a 1 + a 2 = a k （ k 是某个正整数），则2a 1 + d = a 1 + (k -1)d ，a 1即 (k - 2)d = a 1 ，因此必有 k ¹ 2 ，且d =k - 2．这样就有 a = a + (n -1)d = a + n -1a ， n 1 1 k - 2 1í而此时对任意正整数 n ，a +a++ a = a n + n (n -1) d = a + (n -1)a + n (n -1) d 1 2 n 1 2 1 12æ n (n -1) ö = a + (n -1)(k - 2) + d ，确实为{a n } 中的一项．ç 1 çè 2 ø 因此，仅需考虑使 k - 2| a 1 成立的正整数 k 的个数．注意到 2019 为两个素数3 与 673 之积，易知 k - 2 可取-1, 1, 3, 673, 2019 这5 个值，对应得到5 个满足条件的等差数列．二、解答题：本大题共 3 小题，满分 56 分．解答应写出文字说明、证明过程或演算步骤．9.（本题满分 16 分）在椭圆G 中， F 为一个焦点， A , B 为两个顶点．若 FA = 3, FB = 2 ，求 AB 的所有可能值．解：不妨设平面直角坐标系中椭圆 G 的标准方程为 x2y 2+= 1 (a > b > 0) ，并记 c = a 2 b 2a 2 -b 2 ．由对称性，可设 F 为 G 的右焦点．易知 F 到 G 的左顶点的距离为 a +c ，到右顶点的距离为 a - c ，到上、下顶点的距离均为 a ．分以下情况讨论：(1) A , B 分别为左、右顶点．此时a + c = 3, a - c = 2 ，故 AB = 2a = 5 （相应地，b 2= (a + c )(a - c ) = 6 ，G 的方程为4 x 2y 2+ = 1 ）． …………………4 分25 6(2) A 为左顶点，B 为上顶点或下顶点．此时 a + c = 3, a = 2 ，故 c = 1 ，进2 2而 b 2 = a 2 - c 2 = 3 ，所以 AB =a 2 +b 2= 7（相应的 G 的方程为 x + y = 1 ）．4 3…………………8 分(3) A 为上顶点或下顶点， B 为右顶点．此时 a = 3, a - c = 2 ，故 c = 1 ，进2 2而 b 2 = a 2 - c 2 = 8 ，所以 AB =a 2 +b 2 = 17（相应的 G 的方程为 x + y= 1 ）．9 8…………………12 分综上可知， AB 的所有可能值为5, 7, 17 ． …………………16 分10. （本题满分 20 分）设 a , b , c 均大于 1，满足ìïlg a + log b c = 3, ïîlg b + log a c = 4. 求 lg a ⋅ lg c 的最大值．解：设lg a = x , lg b = y , lg c = z ，由 a , b , c >1可知 x , y , z > 0 ．由条件及换底公式知 x + z = 3, y + z= 4 ，即xy + z = 3y = 4x ． y x…………………5 分。

2019年全国数学竞赛试题详细参考答案

中国教育学会中学数学教学专业委员会《数学周报》杯” 2013年全国初中数学竞赛试题参考答案题号-一一 _ 二 _ 三总分1〜56〜1011121314得分评卷人复查人答题时注意：1用圆珠笔或钢笔作答2•解答书写时不要超过装订线. 3.草稿纸不上交.一、选择题(共5小题，每小题6分，满分30分.以下每道小题均给出了代号为 A , B , C , D 的四个选项，其中有且只有一个选项是正确的 .请将正确选项的代号填入题后的括号里.不填、多填或错填都得0分)1.已知实数x , y 满足刍二=3, y 4 - y^3，则-44 y 4的值为().XXx(A ) 7 (B )(C ) 7 "3(D )52 2【答】(A ) 解：因为x 20，y 2 > 0,由已知条件得-1,13244 y 4 乡 3 3-y 2£ -y 2 6 =7.X XX程为t 2 +t-3=0，所以(一W )+ y 2 =-1, (―寸=-3X X2.把一枚六个面编号分别为1, 2, 3, 4, 5, 6的质地均匀的正方体骰子先后投掷2次，若两个正面朝上的编号分别为 m , n ,则二次函数y = x 2 • mx • n 的图象与X 轴有两个不同交点的概率是().(D)所以另解：由已知得: 2 2 2」(一P )2+(—P )—3=0 X X Q 2) + y 2-3 = 0显然 2 2 2 2 2 -y 2，以- 2 ,y 2为根的一元二次方 XX42故 4y 4 二[(- 2)y 2]2 -2XX2 2 22)y =(T) -2 (-3)=7 X12.4 4 4 3［答］（ C ）解：基本事件总数有60 = 36,即可以得到36个二次函数.由题意知；_ =_4n >0,即卩 m 2 >4n .通过枚举知，满足条件的 m, n 有 17 对.363.有两个同心圆，大圆周上有 4个不同的点，小圆周上有可以确定的不同直线最少有（）.2个不同的点，则这6个点 (A ) 6条 (B ) 8 条(C ) 10 条(D ) 12 条【答］（B ）解：如图，大圆周上有4个不同的点A ，B ，C ，D ，两两连线可以确定6条不同的直线；小圆周上的两个点 E ，F 中，至少有一个不是四边形ABCD 的对角线AC 与BD 的交点，则它与A ，B ，C ， D 的连线中，至少有两条不同于 A ，B ，C ，D 的两两连线.从而这 6个点可以确定的直线不少于 8条.当这6个点如图所示放置时，恰好可以确定 8条直线. 所以，满足条件的6个点可以确定的直线最少有8条.4 .已知AB 是半径为1的圆O 的一条弦，且 AB 二a ：：：1 .以AB 为一边在圆O 内作正△ ABC ，点D 为圆O 上不同于点A 的一点，且DB 二AB 二a ， AE 的长为（）.(B) 1（C ）乎【答］（B ）解:女口图，连接 OE ，OA ，OB .设.D =:，贝UECA=120- EAC .11又因为 ABO ABD 60180 -2：-120 -:22所以△ ACE 也△ ABO ，于是AE = OA = 1 .另解：如图，作直径EF ，连结AF ，以点B 为圆心，AB 为半径作。

2019年中国数学奥林匹克完整试题及解析

(因为
C325
≥
max L(x, y)).
(x,y)∈X
下面构造例子说明 C325 是可以取到的最好的常数:
设 S = {1, 2, · · · , 35}, 考虑置换 f = (12 · · · 35), 即:
f (1) = 2, f (2) = 3, · · · , f (34) = 35, f (35) = 1,
极值可以在满足一些对称性的序列 (ai) 上取到. 毋庸置疑，满足题目条件的数列集合是闭集，因此两个
小题中的目标函数（都是连续的）确实能取到最大值.
（1）设序列
(ai)
使
a+b+c+d
取到最大，令
ci
=
ai
+
ai+10
+ ai+20 4
+ ai+30 ，下标模
40
理解.
根
据上一段，ci 满足题目条件，而且（1）中目标函数在序列 (ai) 和 (ci) 上取值相同，因此可以只对具有
2019 年中国数学奥林匹克试题解析
6
注意到: L(x, y) ≤ L(f1(x), f1(y)) + 1, 从而
L(x, y) ≤ L (fk−1(· · · (f1(x))), fk−1(· · · (f1(y)))) + (k − 1) = k ≤ |X| = C325,
所以
m
≥
C325
时总是可以办到的
（解题：）
题 2. 已知：△ABC 中，AD 为角平分线，E 为 AD 上一点，EF 、EG 为 △ABD、△ACD 外接圆切线，F 、G 分别为切点，CF 交 BG 于 J. 过 J 的 BC 平行线交 DF 、DG、DE 于 H、I、K.

第十届全国大学生数学竞赛决赛试题参考答案及评分标准(非数学类)

第十届全国大学生数学竞赛决赛试题参考答案及评分标准（非数学类，2019年3月30日）一、填空题（本题满分30分，每小题6分）1、设函数在点在处连续，则的值为答案：2、设则答案：3、设曲线L是空间区域的表面与平面的交线，则答案：4、设函数由方程确定，其中具有连续二阶偏导数，则答案：5、已知二次型，则的规范形为答案：二、设内三阶连续可导，满足，又设数列满足严格单调减少且计算【解】由于在区间（-1,1）内三阶可导，在处有Taylor公式又，所以分①由于数列严格单调且，则，且为严格单调增加趋于正无穷的数列，注意到，故由Stolz定理及①式，有分分三、设上具有连续导数，且证明：对于成立【证明】令则故函数在上严格单调增加，记的反函数为，则定义在上，且4分于是根据积分中值定理，存在使得分因此注意到则即分四、计算三重积分：，其中【解】采用“先二后一”法，并利用对称性，得其中分用极坐标计算二重积分，得交换积分次序，得分作变量代换:并利用对称性，得所以.分五、之和.【解】级数通项令分其中.因为所以满足解这个一阶线性方程，得由得,故且分六、设A是n阶幂零矩阵,即满足证明:若A的秩为r，且则存在n阶可逆矩阵P其中为r阶单位矩阵. 【证】存在n阶可逆矩阵H,Q，使得因为所以有分对QH作相应分块为则有因此分而所以显然，所以为行满秩矩阵.8分因为使得分令则有分七、设为单调递减的正实数列,收敛，证明：收敛，所以对任意给定，存在自然数，使得当时,有因为单调递减的正数列，所以分注意到当时，有令得到分下面证明：对于任意自然数n，如果满足则有事实上,即得到分利用（2），令可以得到即分又由知，存在自然数，使得分取则当时，有因此分。

2019年高中数学竞赛试题及答案及答案

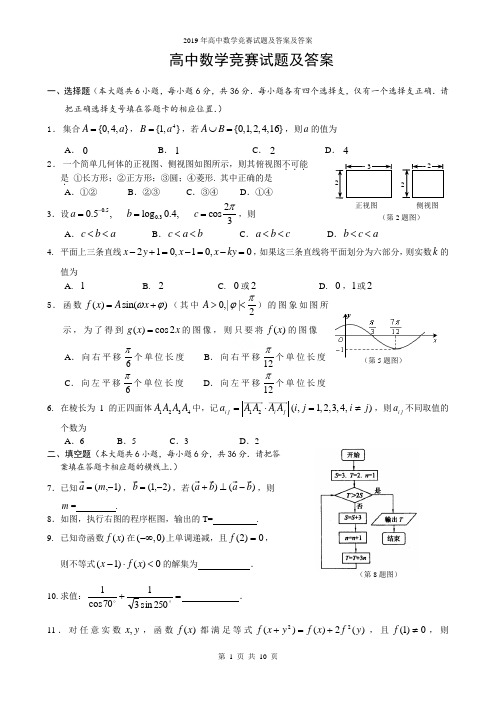
高中数学竞赛试题及答案一、选择题（本大题共6小题，每小题6分，共36分．每小题各有四个选择支，仅有一个选择支正确．请把正确选择支号填在答题卡的相应位置．）1．集合{0,4,}A a =，4{1,}B a =，若{0,1,2,4,16}A B ⋃=，则a 的值为A ．0B ．1C ．2D ．2．一个简单几何体的正视图、侧视图如图所示，则其俯视图不可能．．．是．①长方形；②正方形；③圆；④菱形. 其中正确的是 A ．①② B ．②③ C ．③④ D ．①④ 3．设0.50.320.5,log 0.4,cos3a b c π-===，则A ．c b a <<B ．c a b <<C ．a b c <<D ．b c a <<4. 平面上三条直线210,10,0x y x x ky -+=-=-=，如果这三条直线将平面划分为六部分，则实数k 的值为A . 1B . 2C . 0或2D . 0，1或2 5．函数()sin()f x A x ωϕ=+（其中0,||2A πϕ><）的图象如图所示，为了得到()cos 2g x x =的图像，则只要将()f x 的图像A ．向右平移6π个单位长度 B ．向右平移12π个单位长度 C ．向左平移6π个单位长度 D ．向左平移12π个单位长度6. 在棱长为1的正四面体1234A A A A 中，记12(,1,2,3,4,)i j i j a A A A A i j i j =⋅=≠，则i j a 不同取值的个数为A ．6B ．5C ．3D ．2二、填空题（本大题共6小题，每小题6分，共36分．请把答案填在答题卡相应题的横线上．） 7．已知)1,(-=m a ，)2,1(-=b ，若)()(b a b a -⊥+，则m = .8．如图，执行右图的程序框图，输出的T= . 9. 已知奇函数()f x 在(,0)-∞上单调递减，且(2)0f =，则不等式0)()1(<⋅-x f x 的解集为．10.求值：=+250sin 3170cos 1 ． 11．对任意实数y x ,，函数)(x f 都满足等式)(2)()(22y f x f y x f +=+，且0)1(≠f ，则（第5题图）（第8题图）3侧视图正视图2222=)2011(f .12.在坐标平面内，对任意非零实数m ，不在抛物线()()22132y mx m x m =++-+上但在直线1y x =-+ 上的点的坐标为 .答题卡一、选择题（本大题共6小题，每小题6分，共36分．）二、填空题（本大题共6小题，每小题6分，共36分．）7． 8． 9． 10． 11． 12．三、解答题（本大题共6小题，共78分.解答应写出必要的文字说明、证明过程或演算步骤.） 13.（本小题满分12分）为预防(若疫苗有效已知在全体样本中随机抽取1个，抽到B 组的概率是0.375. （1）求x 的值；（2）现用分层抽样的方法在全部测试结果中抽取360个，问应在C 组中抽取多少个？（3）已知465≥y ,25≥z ,求该疫苗不能通过测试的概率.已知函数x x x f 2sin )12(cos 2)(2++=π．（1）求)(x f 的最小正周期及单调增区间；（2）若),0(,1)(παα∈=f ，求α的值． 15．（本题满分13分）如图，在直三棱柱111C B A ABC -中，21===AA BC AC ，︒=∠90ACB ，G F E ,,分别是AB AA AC ,,1的中点．（1）求证：//11C B 平面EFG ；（2）求证：1AC FG ⊥；（3）求三棱锥EFG B -1的体积.ACBB 1A 1C 1FGE已知函数t t x x x f 32)(22+--=.当∈x ),[∞+t 时，记)(x f 的最小值为)(t q . (1)求)(t q 的表达式；(2)是否存在0<t ，使得)1()(tq t q =？若存在，求出t ；若不存在，请说明理由.已知圆22:228810M x y x y +---=和直线:90l x y +-=，点C 在圆M 上，过直线l 上一点A 作MAC ∆.（1）当点A 的横坐标为4且45=∠MAC 时，求直线AC 的方程；（2）求存在点C 使得45=∠MAC 成立的点A 的横坐标的取值范围.18．（本题满分14分）在区间D 上，若函数)(x g y =为增函数，而函数)(1x g xy =为减函数，则称函数)(x g y =为区间D 上的“弱增”函数．已知函数()1f x =-．（1）判断函数()f x 在区间(0,1]上是否为“弱增”函数，并说明理由；（2）设[)1212,0,,x x x x ∈+∞≠，证明21211()()2f x f x x x -<-；（3）当[]0,1x ∈时，不等式xax +≥-111恒成立，求实数a 的取值范围．参考答案一、选择题：C B A D D C二、填空题：7. 2± 8．29 9. ),2()1,0()2,(+∞--∞10.3 11．2201112. 31(,),(1,0),(3,4)22-- 三、解答题：13. （本题满分12分）解：（1）因为在全体样本中随机抽取1个，抽到B 组的概率0.375，所以375.0200090=+x ， ………………2分即660x =. ………………3分（2）C 组样本个数为y ＋z ＝2000－（673＋77＋660＋90）＝500， ………………4分现用分层抽样的方法在全部测试结果中抽取360个，则应在C 组中抽取个数为360500902000⨯=个. ………………7分（3）设事件“疫苗不能通过测试”为事件M.由（2）知 500y z +=，且,y z N ∈,所以C 组的测试结果中疫苗有效与无效的可能的情况有：（465，35）、（466，34）、（467，33）、……（475，25）共11个. ……………… 9分由于疫苗有效的概率小于90%时认为测试没有通过，所以疫苗不能通过测试时，必须有9.02000660673<++y， …………………10分即1800660673<++y ，解得467<y ，所以事件M 包含的基本事件有：（465，35）、（466，34）共2个. …………………11分所以112)(=M P ，故该疫苗不能通过测试的概率为211. …………………12分14. （本小题满分12分）解：x x x f 2sin )62cos(1)(+++=π…………………1分x x x 2sin 6sin2sin 6cos 2cos 1+-+=ππx x 2sin 212cos 231++= ………………… 2分 1)32sin(++=πx . …………………4分（1）)(x f 的最小正周期为ππ==22T ； …………………5分又由]22,22[32πππππ+-∈+k k x ， …………………6分得)](12,125[Z k k k x ∈+-∈ππππ， …………………7分从而)(x f 的单调增区间为)](12,125[Z k k k ∈+-ππππ． …………………8分（2）由11)32sin()(=++=πααf 得0)32sin(=+πα， …………………9分所以ππαk =+32，62ππα-=k )(Z k ∈． …………………10分又因为),0(πα∈，所以3πα=或65π． …………………12分15. （本题满分13分）解：（1）因为E G 、分别是AC AB 、的中点，所以BC GE //；……1分又BC C B //11，所以GE C B //11； …………2分又⊆GE 平面EFG ，⊄11C B 平面EFG ，所以//11C B 平面EFG ． …………3分（2）直三棱柱111C B A ABC -中，因为︒=∠90ACB ，所以⊥BC 平面C C AA 11； ……………4分又BC GE //，所以⊥GE 平面C C AA 11，即1AC GE ⊥； ……………5分又因为21==AA AC ，所以四边形11A ACC 是正方形，即11AC C A ⊥； ……………6分又F E ,分别是1,AA AC 的中点，所以C A EF 1//，从而有1AC EF ⊥， ……………7分由E GE EF =⋂，所以⊥1AC 平面EFG ，即1AC FG ⊥． ……………8分（3）因为//11C B 平面EFG ，所以111EFC G EFG C EFG B V V V ---==． ……………10分由于⊥GE 平面C C AA 11，所以GE S V EFC EFC G ⋅=∆-1131，且121==BC GE ．…………11分又由于2321114111111=---=---=∆∆∆∆ECC FC A AEF A ACC EFC S S S S S 正方形，……………12分所以21123313111=⋅⋅=⋅=∆-GE S V EFC EFC G ，即211=-EFG B V ． ……………13分16. （本题满分13分）解：（1）t t x x x f 32)(22+--=13)1(22-+--=t t x ． ……………1分①当1≥t 时，)(x f 在∈x ),[∞+t 时为增函数，所以)(x f 在∈x ),[∞+t 时的最小值为t t f t q ==)()(；……………3分②当1<t 时，13)1()(2-+-==t t f t q ； ……………5分综上所述，2(1)()31(1)t t q t t t t ≥⎧=⎨-+-<⎩． ……………6分ACBB 1A 1C 1FGE（2）由（1）知，当0<t 时，13)(2-+-=t t t q ，所以当0<t 时，131)1(2-+-=tt tq ． ……………7分由)1()(t q t q =得：1311322-+-=-+-tt t t ， ……………8分即013334=-+-t t t ， ……………9分整理得0)13)(1(22=+--t t t ， ……………11分解得：1±=t 或253±=t . ……………12分又因为0<t ，所以1-=t .即存在1-=t ，使得)1()(tq t q =成立. ……………13分17. （本题满分14分）解：（1）圆M 的方程可化为：2217(2)(2)2x y -+-=，所以圆心M （2，2），半径r=2. ……1分由于点A 的横坐标为4，所以点A 的坐标为（4，5），即AM =……………2分若直线AC 的斜率不存在，很显然直线AM 与AC 夹角不是45，不合题意，故直线AC 的斜率一定存在，可设AC 直线的斜率为k ，则AC 的直线方程为5(4)y k x -=-，即540kx y k -+-=. ……………3分由于45=∠MAC 所以M 到直线AC 的距离为226||22==AM d ，此时r d <，即这样的点C 存在. ……………4分2=，2=，解得15 5k k =-=或. ……………5分所以所求直线AC 的方程为0255=-+y x 或0215=+-y x . ……………6分 (2)当r AM 2||=时，过点A 的圆M 的两条切线成直角，从而存在圆上的点C （切点）使得45=∠MAC . ……………7分设点A 的坐标为),(y x ，则有⎪⎩⎪⎨⎧=-+=⋅=-+-09172342)2()2(22y x y x ， ……………8分解得⎩⎨⎧==63y x 或⎩⎨⎧==36y x . ……………9分记点)6,3(为P ，点)3,6(为Q ，显然当点A 在线段PQ 上时，过A 的圆的两条切线成钝角，从而必存在圆上的一点C 使得45=∠MAC ；……当点A 在线段PQ 的延长线或反向延长线上时，过A 的圆的两条切线成锐角，从而必不存在圆上的点C 使得45=∠MAC ， …………所以满足条件的点A 为线段PQ 上的点，即满足条件的点的横坐标取值范围是.……14分18．（本题满分14分）解：（1）由()1f x =-可以看出，在区间(0,1]上，()f x 为增函数. ………………1分又11()(1f x x x ===3分显然)(1x f x在区间(0,1]∴ ()f x 在区间(0,1]为“弱增”函数. ………………4分（2）21()()f x f x -===.…6分[)1212,0,,x x x x ∈+∞≠,∴111≥+x ，112≥+x ，21121>+++x x ，即2>，………………8分21()()f x f x ∴-2112x x <-. ………………9分（3）当0x =时,不等式xax +≥-111显然成立. ………………10分“当(]0,1x ∈时，不等式xax +≥-111恒成立”等价于“ 当(]0,1x ∈时，不等式)111(1xx a +-≤即)(1x f x a ≤恒成立” . ………………11分也就等价于:“ 当(]0,1x ∈时， min )](1[x f xa ≤成立” . ………………12分由(1)知1()f x x 在区间(0,1]上为减函数, 所以有221)1()](1[min -==f x f x . ……………13分 ∴221-≤a ，即221-≤a 时，不等式xax +≥-111对[]0,1x ∈恒成立. ……………14分。

2019年全国高中数学联赛试卷及答案-10页文档资料

2019年全国高中数学联合竞赛试卷第一试一、选择题本题共有6小题，每题均给出（A ）、（B ）、（C ）、（D ）四个结论，其中有且仅有一个是正确的，请将正确答案的代表字母填在题后的括号内，每小题选对得6分；不选、选错或选出的代表字母超过一个（不论是否写在括号内），一律得0分。

1．给定公比为q (q ≠1)的等比数列{a n }，设b 1=a 1+a 2+a 3, b 2=a 4+a 5+a 6,…,b n =a 3n -2+a 3n -1+a 3n ,…，则数列{b n } 【答】（） (A ) 是等差数列 (B ) 是公比为q 的等比数列 (C ) 是公比为q 3的等比数列 (D ) 既非等差数列也非等比数列2．平面直角坐标系中，纵、横坐标都是整数的点叫做整点，那么，满足不等式(|x |-1)2+(|y |-1)2＜2的整点(x ,y )的个数是【答】（） (A ) 16 (B ) 17 (C ) 18 (D ) 253．若(log 23)x -(log 53)x ≥(log 23)y --(log 53)y-，则【答】（）(A ) x -y ≥0 (B ) x +y ≥0 (C ) x -y ≤0 (D ) x +y ≤0 4．给定下列两个关于异面直线的命题：命题Ⅰ：若平面α上的直线a 与平面β上的直线b 为异面直线，直线c 是α与β的交线，那么，c 至多与a ,b 中的一条相交；命题Ⅱ：不存在这样的无穷多条直线，它们中的任意两条都是异面直线。

那么【答】（） (A ) 命题Ⅰ正确，命题Ⅱ不正确 (B ) 命题Ⅱ正确，命题Ⅰ不正确 (C ) 两个命题都正确 (D ) 两个命题都不正确5．在某次乒乓球单打比赛中，原计划每两名选手恰比赛一场，但有3名选手各比赛了2场之后就退出了，这样，全部比赛只进行了50场。

那么，在上述3名选手之间比赛的场数是【答】（） (A ) 0 (B ) 1 (C ) 2 (D ) 36．已知点A (1,2)，过点(5,-2)的直线与抛物线y 2=4x 交于另外两点B ,C ，那么，△ABC 是(A ) 锐角三角形 (B ) 钝角三角形 (C ) 直角三角形 (D ) 不确定【答】（）二、填空题（本题满分54分，每小题9分）本题共有6小题，要求直接将答案写在横线上。

第十届全国大学生数学竞赛决赛参考答案

n!1
阅卷人
1; 又设数列
), 严格单调减少且
lim an = 0. 计算
2 lim nan n!1
解. 由于 f (x ) 在区间 ( 1; 1) 内三阶连续可导, f (x ) 在点 x = 0 处有 Tayor 公式 f (x ) = f (0) + f 0 (0)x + f 00 (0) 2 f 000 (0) 3 x + x + o(x 3 ); 2 3! 1, 所以 1 3 x + o(x 3 ): 6 (1)
(8 分) (10 分)
(11 分)
得
分
阅卷人
n!1
七、 (本题满分 11 分) 设 fun g1 n=1 为单调递减的正实数列, 1 X lim un = 0, fan g1 为一实数列 , 级数 an un 收敛, 证明: n=1
n=1 n!1
lim (a1 + a2 +
+ an )un = 0:
分
阅卷人座位号证明. 令 y = x
1 1 , 则 y0 = 1 + > 0. 故函数 y (x ) 在 [˛; ˇ ] 上严格单调增加. 记 y (x ) 的反 nx nx 2 Ä 1 1 函数为 x (y ), 则 x (y ) 定义在 ˛ ;ˇ 上, 且 n˛ nˇ x 0 (y ) = 1 y 0 (x ) = 1 > 0: 1 1 + nx 2
密封线答题时不要超过此线
得
分
一、填空题 (本题满分 30 分, 共 5 小题, 每小题 6 分)
阅卷人 8p ˆ < 1 ˆ : Z
准考证号
(1) 设函数 y =

2019年全国初中数学联赛试题及详解

2019年全国初中数学联合竞赛试题及详解第一试一、选择题：（本题满分42分，每小题7分）1. 若,,a b c 均为整数且满足1010()()1a b a c -+-=，则||||||a b b c c a -+-+-= （ B ）A ．1.B ．2.C ．3.D ．4.解：由已知可推得011a b b c a c -=⎧⇒-=±⎨-=±⎩ 或 110a b b c a c -=±⎧⇒-=±⎨-=⎩，分别代入即得。

2．若实数,,a b c 满足等式23||6a b =，9||6a b c =，则c 可能取的最大值为（ C ）A ．0.B ．1.C ．2.D ．3.解：由已知，6492(23)15121512c a b a b b b ==-=-≤，∴2c ≤.3．若b a ,是两个正数，且,0111=+-+-ab b a 则（ C ） A ．103a b <+≤. B ．113a b <+≤. C ．413a b <+≤. D ．423a b <+≤. 解：当a b =时，可计算得23a b ==，从而43a b +=。

观察4个选项，只能选C. 4．若方程2310x x --=的两根也是方程420x ax bx c +++=的根，则2a b c +-的值为（ A ）A ．－13.B ．－9.C ．6.D ． 0.解：由已知：42x ax bx c +++一定能被231x x --整除。

∵4222(31)(310)[(333)(10)]x ax bx c x x x x a a b x a c +++=--+++++++++∴(333)(10)0a b x a c +++++=，故3330213100a b a b c a c ++=⎧⇒+-=-⎨++=⎩5．在△ABC 中，已知︒=∠60CAB ，D ，E 分别是边AB ，AC 上的点，且︒=∠60AED ，CE DB ED =+，CDE CDB ∠=∠2,则=∠DCB ( B )A ．15°.B ．20°.C ．25°.D ．30°.解：如图，由已知，ADE 是正三角形。

2019年全国高中数学联赛试题及答案详解(B卷)

2019年全国高中数学联合竞赛一试（B 卷）参考答案及评分标准说明：1. 评阅试卷时，请依据本评分标准. 填空题只设8分和0分两档；其他各题的评阅，请严格按照本评分标准的评分档次给分，不得增加其他中间档次.2. 如果考生的解答方法和本解答不同，只要思路合理、步骤正确，在评卷时可参考本评分标准适当划分档次评分，解答题中第9小题4分为一个档次，第10、11小题5分为一个档次，不得增加其他中间档次．一、填空题：本大题共8小题，每小题8分，满分64分．1. 已知实数集合{1,2,3,}x 的最大元素等于该集合的所有元素之和，则x 的值为．答案：3-．解：条件等价于1,2,3,x 中除最大数以外的另三个数之和为0．显然0x <，从而120x ++=，得3x =-．2. 若平面向量(2,1)m a =-与1(21,2)m m b +=-垂直，其中m 为实数，则a 的模为．答案解：令2m t =，则0t >．条件等价于(1)(1)20t t t ⋅-+-⋅=，解得3t =．因此a=．3. 设,(0,)a b p Î，cos ,cos a b 是方程25310x x --=的两根，则sin sin a b 的值为．答案：5．解：由条件知31cos cos ,cos cos 55a b a b +==-，从而222(sin sin )(1cos )(1cos )a b a b =--22221cos cos cos cos a b a b=--+2222437(1cos cos )(cos cos )5525a b a b æöæö÷çç=+-+=-=÷çç÷ççèøè．又由,(0,)a b p Î知sin sin 0a b >，从而sin sin 5a b =． 4. 设三棱锥P ABC -满足3,2PA PB AB BC CA =====，则该三棱锥的体积的最大值为．答案：3．解：设三棱锥P ABC -的高为h ．取M 为棱AB 的中点，则h PM £==．当平面PAB 垂直于平面ABC 时，h 取到最大值．此时三棱锥P ABC -的体积取到最大值11333ABC S D ⋅==．5. 将5个数2,0,1,9,2019按任意次序排成一行，拼成一个8位数（首位不为0），则产生的不同的8位数的个数为．答案：95．解：易知2,0,1,9,2019的所有不以0为开头的排列共有44!96´=个．其中，除了(2,0,1,9,2019)和(2019,2,0,1,9)这两种排列对应同一个数20192019，其余的数互不相等．因此满足条件的8位数的个数为96195-=．6. 设整数4n >，(1)n x +的展开式中4n x -与xy 两项的系数相等，则n 的值为．答案：51．解：注意到0(1)C 1)nnr n r r nr x x -=+=å．其中4n x -项仅出现在求和指标4r =时的展开式444C 1)n n x-中，其4n x -项系数为44(1)(2)(3)(1)C 24n n n n n ----=．而xy 项仅出现在求和指标1r n =-时的展开式11C 1)n n nx --⋅中，其xy 项系数为12331C C 4(1)(1)2(1)(2)n n n n n n n n ----⋅-=---．因此有3(1)(2)(3)(1)2(1)(2)24n n n n n n n n ----=---．注意到4n >，化简得33(1)48n n --=-，故只能是n 为奇数且348n -=．解得51n =．7. 在平面直角坐标系中，若以(1,0)r +为圆心、r 为半径的圆上存在一点(,)a b 满足24b a ³，则r 的最小值为．答案：4．解：由条件知222(1)a r b r --+=，故22224(1)2(1)(1)a b r a r r a a £=---=---．即22(1)210a r a r --++£．上述关于a 的一元二次不等式有解，故判别式2(2(1))4(21)4(4)0r r r r --+=-³，解得4r ³．经检验，当4r =时，(,)(3,a b =满足条件．因此r 的最小值为4．8. 设等差数列{}n a 的各项均为整数，首项12019a =，且对任意正整数n ，总存在正整数m ，使得12n m a a a a +++=．这样的数列{}n a 的个数为．答案：5．解：设{}n a 的公差为d ．由条件知12k a a a +=（k 是某个正整数），则 112(1)a d a k d +=+-，即1(2)k d a -=，因此必有2k ¹，且12ad k =-．这样就有1111(1)2n n a a n d a a k -=+-=+-，而此时对任意正整数n ，12111(1)(1)(1)22n n n n n a a a a n d a n a d --+++=+=+-+ 1(1)(1)(2)2n n a n k d æö-÷ç=+--+÷ç÷çèø，确实为{}n a 中的一项．因此，仅需考虑使12|k a -成立的正整数k 的个数．注意到2019为两个素数3与673之积，易知2k -可取1,1,3,673,2019-这5个值，对应得到5个满足条件的等差数列．二、解答题：本大题共3小题，满分56分．解答应写出文字说明、证明过程或演算步骤．9.（本题满分16分）在椭圆G 中，F 为一个焦点，,A B 为两个顶点．若3,2FA FB ==，求AB 的所有可能值．解：不妨设平面直角坐标系中椭圆G 的标准方程为22221(0)x y a b a b+=>>，并记c =F 为G 的右焦点．易知F 到G 的左顶点的距离为a c +，到右顶点的距离为a c -，到上、下顶点的距离均为a ．分以下情况讨论：(1) ,A B 分别为左、右顶点．此时3,2a c a c +=-=，故25AB a ==（相应地，2()()6b a c a c =+-=，G 的方程为2241256x y +=）． …………………4分(2) A 为左顶点，B 为上顶点或下顶点．此时3,2a c a +==，故1c =，进而2223b a c =-=，所以AB ==G 的方程为22143x y +=）． …………………8分 (3) A 为上顶点或下顶点，B 为右顶点．此时3,2a a c =-=，故1c =，进而2228b a c =-=，所以AB ==G 的方程为22198x y +=）．…………………12分综上可知，AB的所有可能值为5,． …………………16分10. （本题满分20分）设,,a b c 均大于1，满足lg log 3,lg log 4.b a a c b c ì+=ïïíï+=ïî求lg lg a c ⋅的最大值．解：设lg ,lg ,lg a x b y c z ===，由,,1a b c >可知,,0x y z >．由条件及换底公式知3,4z zx y y x+=+=，即34xy z y x +==．…………………5分由此，令3,4(0)x t y t t ==>，则241212z x xy t t =-=-．其中由0z >可知(0,1)t Î． …………………10分因此，结合三元平均值不等式得2lg lg 312(1)18(22)a c xz t t t t t ==⋅-=⋅-33(22)2161818333t t t æöæö++-÷çç£⋅=⋅=÷çç÷ççèèø．当22t t =-，即23t =（相应的,,a b c 分别为8833100,10,10）时，lg lg a c 取到最大值163． …………………20分11. （本题满分20分）设复数数列{}n z 满足：11z =，且对任意正整数n ，均有2211420n n n n z z z z ++++=．证明：对任意正整数m ，均有123m z z z +++<．证明：归纳地可知*0()n z n N ¹Î．由条件得2*114210()n n n n z z n z z N ++æöæö÷çç÷++=Îçç÷çç÷èøèø，解得*11()4N n n z n z +-=Î． …………………5分因此1112n n nnz z z z ++===，故*11111()22N n n n z z n --=⋅=Î． ①进而有*11111()22N n n n n n n n z z z z n z ++-+=⋅+==Î． ②…………………10分当m 为偶数时，设*2()N m s s =Î．利用②可得122122122111123sm k k k k k k k k z z z z z z z ¥¥---===+++£+<+==ååå． …………………15分当m 为奇数时，设21()N m s s =+Î．由①、②可知21212221211112322s k k s s k k s k s z z z ¥¥+---=+=+=<==+⋅åå，故1221221212113s m k k s k k k k z z z z z z z z ¥-+-==æö÷ç+++£++<+=÷ç÷çèøåå．综上，结论获证． …………………20分2019年全国高中数学联合竞赛加试（B 卷）参考答案及评分标准说明：1．评阅试卷时，请严格按照本评分标准的评分档次给分．2．如果考生的解答方法和本解答不同，只要思路合理、步骤正确，在评卷时可参考本评分标准适当划分档次评分，10分为一个档次，不得增加其他中间档次．一、（本题满分40分）设正实数12100,,,a a a 满足101(1,2,,50)i i a a i -³=．记112(1,2,,99)k k kka x k a a a +==+++．证明：29912991x x x £．证明：注意到12100,,,0a a a >．对1,2,,99k =，由平均值不等式知121210kk k k a a a a a a æöç<£çç+++èø， ……………10分从而有9999299112991111212kk k k k k k k ka k x x x a a a a a a a ++==æö÷ç÷=£ç÷÷ç+++èø ． ①………………20分记①的右端为T ，则对任意1,2,,100i =，i a 在T 的分子中的次数为1i -，在T 的分母中的次数为100i -．从而10121005050210121012(101)101101101111ii i i i i i i i i i ia T a a a a -------===æö÷ç÷===ç÷ç÷èø ．………………30分又1010(1,2,,50)i i a a i -<£=，故1T £，结合①得29912991x x x T ££． ………………40分二、（本题满分40分）求满足以下条件的所有正整数n ：(1) n 至少有4个正约数；(2) 若12k d d d <<< 是n 的所有正约数，则21321,,,k k d d d d d d ---- 构成等比数列．解：由条件可知4k ≥，且3212112kk k k d d d d d d d d -----=--． ………………10分易知112231,,,k k k n nd d n d d d d --====，代入上式得3222231n n d d d n n d d d --=--，化简得223223()(1)d d d d -=-． ………………20分由此可知3d 是完全平方数．由于2d p =是n 的最小素因子，3d 是平方数，故只能23d p =． ………………30分从而序列21321,,,k k d d d d d d ---- 为23212,1,,,k k p p p p p p p ------ ，即123,,,,k d d d d 为21,1,,,k p p p - ，而此时相应的n 为1k p -．综上可知，满足条件的n 为所有形如a p 的数，其中p 是素数，整数3a ≥． ………………40分三、（本题满分50分）如图，点,,,,A B C D E在一条直线上顺次排列，满足BC CD ==，点P 在该直线外，满足PB PD =．点,K L 分别在线段,PB PD 上，满足KC 平分BKE ，LC 平分ALD ．证明：,,,A K L E 四点共圆．（答题时请将图画在答卷纸上）证明：令1,(0)AB BC CD t ===>，由条件知2DE t =．注意到180BKE ABK PDE DEK < = < - ，可在CB 延长线上取一点A ¢，使得A KE ABK A BK ¢¢ = = ． ………………10分此时有A BK A KE ∽¢¢D D ，故A B A K BKA K A E KE¢¢==¢¢． ………………20分又KC 平分BKE ，故211BK BC t KE CE t t t===++．于是有 22112A B A B A K BK AB A E A K A E KE t t AEæö¢¢¢÷ç=⋅===÷ç÷ç¢¢¢èø++． …………30分由上式两端减1，得BE BEA E AE=¢，从而A A ¢=．因此AKE A KE ABK ¢ = = ．同理可得ALE EDL = ．而ABK EDL = ，所以AKE ALE = ．因此,,,A K L E 四点共圆． ………………50分四、（本题满分50分）将一个凸2019边形的每条边任意染为红、黄、蓝三种颜色之一，每种颜色的边各673条．证明：可作这个凸2019边形的2016条在内部互不相交的对角线将其剖分成2017个三角形，并将所作的每条对角线也染AA (为红、黄、蓝三种颜色之一，使得每个三角形的三条边或者颜色全部相同，或者颜色互不相同．证明：我们对5n ≥归纳证明加强的命题：如果将凸n 边形的边染为三种颜色,,a b c ，并且三种颜色的边均至少有一条，那么可作满足要求的三角形剖分． ………………10分当5n =时，若三种颜色的边数为1，1，3，由对称性，只需考虑如下两种情形，分别可作图中所示的三角形剖分．若三种颜色的边数为1，2，2，由对称性，只需考虑如下三种情形，分别可作图中所示的三角形剖分．………………20分假设结论对(5)n n ≥成立，考虑1n +的情形，将凸1n +边形记为121n A A A + ．情形1：有两种颜色的边各只有一条．不妨设,a b 色边各只有一条．由于16n +≥，故存在连续两条边均为c 色，不妨设是111,n n n A A A A ++．作对角线1n A A ，并将1n A A 染为c 色，则三角形11n n A A A +的三边全部同色．此时凸n 边形12n A A A 的三种颜色的边均至少有一条，由归纳假设，可对其作符合要求的三角形剖分．………………30分情形2：某种颜色的边只有一条，其余颜色的边均至少两条．不妨设a 色边只有一条，于是可以选择两条相邻边均不是a 色，不妨设111,n n n A A A A ++均不是a 色，作对角线1n A A ，则1n A A 有唯一的染色方式，使得三角形11n n A A A +的三边全部同色或互不同色．此时凸n 边形12n A A A 的三种颜色的边均至少有一条，由归纳假设，可对其作符合要求的三角形剖分． ………………40分情形3：每种颜色的边均至少两条．作对角线1n A A ，则1n A A 有唯一的染色方式，使得三角形11n n A A A +的三边全部同色或互不同色．此时凸n 边形12n A A A 的三种颜色的边均至少有一条，由归纳假设，可对其作符合要求的三角形剖分．综合以上3种情形，可知1n +的情形下结论也成立．由数学归纳法，结论获证． ………………50分。

2019年中国数学奥林匹克完整试题及解析

题 5. 数列 {an } 定义如下: 正整数 a1 > 1, an+1 = an + P (an ), n ≥ 1, 其中, P (x) 表示正整数 x 的最大素因子. 证明: 数列 {an } 中有完全平方数.
题 6. 是否存在正实数 a1 , a2 , · · · , a19 ，使得多项式 P (x) = x20 + a19 x19 + · · · + a1 x + a0 无实数根，但是任意调换两个系数 ai , aj 形成的新多项式都有实根.
（1）设序列 (ai ) 使 a + b + c + d 取到最大，令 ci = 根
，下标模 40 理解.
据上一段， ci 满足题目条件，而且（1）中目标函数在序列 (ai ) 和 (ci ) 上取值相同，因此可以只对具有
周期 10 的序列考虑这个最大值. 此时 a = b = c = d.
a20+k = − k (0 ≤ k ≤ 10), a30+k = a40 − k = − − k (0 ≤ k ≤ 5)
时取等.
（解题人：龚固）
题 2. 已知： △ABC 中， AD 为角平分线， E 为 AD 上一点， EF 、EG 为 △ABD 、△ACD 外接圆切线， F 、G 分别为切点， CF 交 BG 于 J . 过 J 的 BC 平行线交 DF 、DG 、DE 于 H 、I 、K .
(a29+k + a41 − k ) + (a15 + a35 )
≥ (x − 2k) + (x − 2k) + (x − 18 − 2k) + (x − 20)

2019年全国高中数学联赛试题及解答

全国高中数学联合竞赛试题（A 卷）一试一、填空题（本大题共8小题，每小题8分，共64分）1. 若正数,a b 满足()2362log 3log log a b a b +=+=+，则11a b+的值为________．答案：设连等式值为k ，则232,3,6k k ka b a b --==+=，可得答案108分析：对数式恒等变形问题，集训队讲义专门训练并重点强调过2. 设集合3|12b a b a ⎧⎫+≤≤≤⎨⎬⎩⎭中的最大元素与最小你别为,M m ，则M m -的值为______．答案：33251b a +≤+=，33b a a a+≥+≥，均能取到，故答案为5-分析：简单最值问题，与均值、对勾函数、放缩有关，集训队讲义上有类似题 3. 若函数()21f x x a x =+-在[0,)+∞上单调递增，则实数a 的取值范围是______．答案：零点分类讨论去绝对值，答案[]2,0-分析：含绝对值的函数单调性问题，集训队讲义专门训练并重点强调过4. 数列{}n a 满足12a =，()()*1221n n n a a n N n ++=∈+，则2014122013a a a a =+++______．答案：()1221n n n aa n ++=+，迭乘得()121n n a n -=+，()212232421n n S n -=+⨯+⨯+++，乘以公比错位相减，得2n n S n =，故答案为20152013．分析：迭乘法求通项，等差等比乘积求前n 项和，集训队讲义专门训练并重点强调过5. 正四棱锥P ABCD -中，侧面是边长为1的正三角形，,M N 分别是边,AB BC 的中点，则异面直线MN与PC 之间的距离是________．答案：OB 为公垂线方向向量，故距离为12OB =分析：异面直线距离，也可以用向量法做，集训队讲义专门练并重点强调过6. 设椭圆Γ的两个焦点是12,F F ，过点1F 的直线与Γ交于点,P Q ．若212PF F F =，且1134PF QF =，则椭圆Γ的短轴与长轴的比值为________．答案：不妨设焦点在x 轴（画图方便），设114,3PF QF ==，焦距为2c ，224a c =+，可得△2PQF 三边长为7,21,2c c +，过2F 作高，利用勾股可得5c =．分析：椭圆中常规计算，与勾股定理、解三角形、斯特瓦尔特等有关，集训队讲义训练过相关7. 设等边三角形ABC 的内切圆半径为2，圆心为I ．若点P 满足1PI =，则△APB 与△APC 的面积之比的最大值为________．答案：sin sin APB APC S PABS PAC ∠=∠，又两角和为60最大，即AP 与(),1I 切于对称轴右侧2分析：平面几何最值、面积、三角函数、轨迹8. 设,,,A B C D 是空间中四个不共面的点，以12的概率在每对点之间连一条边，任意两点之间是否连边是相互独立的，则,A B 之间可以用空间折线（一条边或者若干条边组成）连结的概率为_______．答案：总连法64种，按由A 到B 最短路线的长度分类．长度为1，即AB 连其余随意，32种；长度为2，即AB 不连，ACB 或ADB 连，其余随意，ACB 连8种，故共88214+-=种（一定注意,ACB ADB 同时连被算了2次，根据CD 是否连有2种情形）；长度为3，两种情形考虑ACDB ，ACDB 连、,,AB CB AD 均不连只有1种，故连法为2种；综上，答案483644=分析：组合计数，分类枚举，难度不大但容易算错，集训队讲义训练过类似题目二、解答题（本大题共3小题，共56分）9. （本题满分16分）平面直角坐标系xOy 中，P 是不在x 轴上的一个动点，满足条件：过P 可作抛物线24y x =的两条切线，两切点连线P l 与PO 垂直．设直线P l 与直线PO ，x 轴的交点分别为,Q R ．（1）证明：R 是一个定点；（2）求PQQR的最小值．答案：（1）设(),P a b ，()()1122,,,A x y B x y ，0,0a b ≠≠，()11:2PA yy x x =+，()22:2PB yy x x =+ 故,A B 两点均适合方程()2by a x =+，利用垂直，可得2a =-，故交点为定点()2,0（2）∵2a =-，故,2PO PR b bk k =-=-，设OPR α∠=，则α为锐角，1tan PQ QR α=，利用两角差的正切公式，可得282PQ b QR b+=≥．分析：涉及圆锥曲线切点弦方程、两直线夹角公式、不等式求最值，集训队讲义专门训练并重点过10. （本题满分20分）数列{}n a 满足16a π=，()()*1arctan sec n n a a n N +=∈．求正整数m ，使得121sin sin sin 100m a a a ⋅⋅⋅=．答案：由反函数值域，知,22n a ππ⎛⎫∈- ⎪⎝⎭，2222132tan sec tan 1tan 3n n n n a a a +-==+==，1212112122311tan tan tan tan tan tan tan sin sin sin sec sec sec tan tan tan tan m m m m m m a a a a a a a a a a a a a a a a a ++⋅⋅⋅=⋅=⋅==故3333m =分析：涉及简单反三角函数、数列通项公式求法，集训队讲义对类似题目进行过训练11. （本题满分20分）确定所有的复数α，使得对任意复数()121212,,1,z z z z z z <≠，均有()()221122z z z z αααα++≠++．答案：转换命题为计算存在12,z z 使得相等时的充要条件存在12,z z 使得相等，记()()2f z z z αα=++，()()()()()1212121220f z f z z z z z z z αα-=++-+-=，则()()()1212122z z z z z z αα-=-++-，故12122222z z z z a ααα=++≥-->-，故2α<；若2α<，令12,22z i z i ααββ=-+=--，其中012αβ<<-，则12z z ≠，122i ααββ-±≤-+<，计算121212,2,2z z z z i z z i αββ+=--=-=-并代入，知()()12f z f z =．综上，满足条件的α为,2Z αα∈≥二试一、（本题满分40分）设实数,,a b c满足1a b c++=，0abc>．求证：14ab bc ca++<．a b c≥≥>，则1a≥1c≤．)ab bc ca c++-+⎭12c-，故有()()111122c c cc cc c⎛---≤-+-⎭⎝⎭由于1110,3333c-≥+≥>310c->，故原不等式成立．方法2：不妨设0a b c≥≥>，则13a≥c，设()()1f b ab bc ca ab c c=++=+-，()f b递增f⇔，()())()1f b ab a b a b⎛'=--=-⎝，()010f b'≥⇔≥⇔≤≥故()f b a；题目转化为21ac+=，a c≥，记()()222212g a a ac a a a=+-=+--()()262621g a a a⎫'=-+=-⎪⎭，由于13a≥1=，得1532a=，115,332a⎛⎫∈ ⎪⎝⎭时g'151,322⎫⎪⎝⎭时()g a在13或12max1124g g⎛⎫==⎪⎝⎭分析：一道偏函数化的不等式题，可以将其放缩为一元函数，也可以拿导数与调整法很快做出来，集训队讲义上两种方法都训练过．二、（本题满分40分）在锐角三角形ABC中，60BAC∠≠，过点,B C分别作三角形ABC的外接圆的切线,BD CE，且满足BD CE BC==．直线DE与,AB AC的延长线分别交于点,F G．设CF与BD交于点M，CE与BG交于点N．证明：AM AN=．答案：设△ABC三边为,,a b c，则BD CE a==，先计算AM，∵,BFD ABC BDF DBC BAC∠=∠∠=∠=∠，∴△BFD∽△CBA．由比例可知acDFb=，故BM BC bBDDF c==，故abBMb c=+，故由余弦定理知()2222cosab abAM c c A Bb c b c⎛⎫=+-⋅+⎪++⎝⎭222cosab abcc Cb c b c⎛⎫=++⎪++⎝⎭，整理可得此式关于,b c对称故可知22AM AN=分析：由于一旦,,a b c三边确定则图形固定，所以通过相似、比例、余弦定理计算的思路比较显然GF ED三、（本题满分50分）设{}1,2,3,,100S =．求最大的整数k ，使得S 有k 个互不相同的非空子集，具有性质：对这k 个子集中任意两个不同子集，若它们的交非空，则它们交集中的最小元素与这两个子集中的最大元素均不相同．答案：一方面，取包含1的、至少含2个元素的所有子集，共9921-个，显然满足题意；另外归纳证对于{}1,2,3,,S n =，任取()123n n -≥个子集，均存在两个的交集中最小的等于某个中最大的当3n =时，将7个非空子集分为三类：{}{}{}31,32,3，{}{}21,2，{}{}11,2,3．任取四个必有两个同类．假设n k =时命题成立，当1n k =+时，如果取出的2k 个子集中至少有12k -个不含1k +，利用归纳假设知成立；如果不含1k +的不足12k -，则至少有121k -+个含有1k +，而S 含有1k +的子集共2k 个，可以配成12k - 对，使得每对中除了公共元素1k +外，其余恰为1到n 的互补子集，这样，如果选出121k -+个，则必有两个除1k +外不交，故命题成立．综上，k 的最大值为9921-．分析：集合中的组合最值问题，比较常规的一道题，类似感觉的题集训队讲义在组合中的归纳法中有过四、（本题满分50分）设整数122014,,,x x x 模2014互不同余，整数122014,,,y y y 模2014也互不同余．证明：可将122014,,,y y y 重新排列为122014,,,z z z ，使得112220142014,,,x z x z x z +++模4028互不同余．答案：不妨设()mod 2014i i x y i ≡≡，1,2,,2014i =．下面对i y 序列进行1007次调整从而构成i z 序列：若i i x y +与10071007i i x y +++模4028不同余，则1007,i i y y +不调整；否则，交换1007,i i y y +位置，1,2,,2014i =．下证，进行1007次调整后，得到的i z 序列一定满足条件．任意挑选一列()1,2,,1007i i x z i +=，只需证其与10071007i i x z +++、()1,2,,1007,j j x z j j i +=≠、10071007j j x z +++模4028不同余即可由i z 构造方法，i i x z +与10071007i i x z +++不同余是显然的，因为不可能调整前后均同余，故只需看另两个；首先，对于不同的,i j ，2i 与2j 模4028不同余，否则会导致()mod 2014i j ≡．若,i j y y 均未调整，则()2mod 2014i i x z i +≡，()100710072mod 2014j j j j x z x z j +++≡+≡，故成立；若,i j y y 均已调整，则()21007mod 2014i i x z i +≡+，()1007100721007mod 2014j j j j x z x z j +++≡+≡+，故成立；若只有一个被调整过，不妨设i y 未调整、j y 已调整，则()2mod 2014i i x z i +≡， ()1007100721007mod 2014j j j j x z x z j +++≡+≡+，若()4028|21007i j --，则()1007|i j -，矛盾，故同样成立．综上，构造的i z 序列满足条件．全国高中数学联赛试题及解答2014高中联赛试题分析从试题类型来看，今年代数、几何、数论、组合4部分所占的比例为：代数37.3%，几何26.7%，数论16.7%，组合19.3%．这方面和历年情况差不多，但具体的知识点差别极大．一试第7题填空题可谓出人意表，虽然解答是用三角函数的方法处理的，对比历年试题，这题毫无疑问也是顶替了三角函数的位置．但本题却是一道彻头彻尾的平面几何题．从图中不难看出，最值情况在相切时取到，剩下的只是利用三角函数处理了一下计算上的问题．其余填空题中，第1~6题和往年出题风格类似，第8题概率计算略显突兀，本题几乎不需要用到计数的技巧，而是用单纯枚举的方法即可解决．放在填空题最后一题的位置不免显得难度不够．一试三道解答题中，第9题和第10题均不太难，所考知识点也和往年类似，无需多说．第11题又再次爆了冷门，考了一道复数问题．联赛已经多年没有考复数的大题了，许多学生都没有准备．可以说，这次一下戳中了学生的罩门．相信本题最终的得分率不容乐观．而本次试题中最特殊的要数加试中的平面几何题了．一反从1997年开始保持到如今的惯例，没有将平面几何题放在加试的第一题．而且本题实则为《中等数学》2012年第12期中的数学奥利匹克高中训练题中的原题，这无疑又让此题失色不少．今年的加试第一题放了一道不等式问题，虽然近几年不等式考察得较少，但是不等式一直是数学竞赛中的热门，在历年联赛中多有出现．考虑到本题难度并不大，放在联赛加试第一题还是非常合适的．加试第三题组合最值问题的出题风格一如既往，可以从很极端的情况下猜出答案，再进行证明．值得全国高中数学联赛试题及解答一提的是本题题干描述有歧义，最后一句“则它们交集中的最小元素与这两个子集中的最大元素均不相同”中，记最小元素为a ，两个最大元素为b 和c ．本句话中到底是指a 、b 、c 这3个数互不相同还是指a b ≠且a c ≠，无疑是容易让人误解的．希望今后联赛试题中能避免出现这种情况．加试第四题虽说考察的是数论中的同余知识，但更多考察的是构造法技巧，这也符合联赛加试中试题综合各方面知识的出题思想．从难度上来说本题难度不算太大，只要能从较小的数开始构造并寻找规律，找出2014的构造并不显得困难．但本题的出题背景无疑和以下题目相关：“n 为给定正整数，()122,,,n x x x 和()122,,,n y y y 均为1~2n 的一个排列，则112222,,,n n x y x y x y +++这2n 个数不可能模2n 互不同余．” 总的说来，本次联赛考察的知识点和往年比差别较大，但从试卷难度来说，和前两年是相当的．预计今年联赛的分数线可能比去年略低．。

2019年全国高中数学联赛试题及答案详解(B卷)

3 22 s-1
=
¥ k=s+1
3 22k-1
¥
=
k=s+1
z2k-1 + z2k
，
故
å å z1 + z2 ++ zm
£
æçççè
k
s =1
z2k-1 + z2k ÷ö÷÷ø+
z2 s+1
¥
<
k =1
z2k-1 + z2k
=
2
3 3
．
综上，结论获证．
…………………20 分
2019 年全国高中数学联合竞赛加试（B 卷）参考答案及评分标准
3. 设 a, b Î (0, p) ，cosa, cosb 是方程 5x2 -3x -1= 0 的两根，则 sin asin b 的
值为
．
答案：
7 5
．
解：由条件知 cosa + cosb = 3 , cosa cos b = -1 ，从而
5
5
(sin a sin b)2 = (1-cos2a)(1- cos2 b) = 1- cos2a - cos2 b + cos2a cos2 b
=
(-1)n-32n(n -1)(n
- 2)
．
因此有
n(n
-1)(n 24
2)(n
-
3)
=
(-1)n-3
2n(n
-1)(n
-
2)
．注意到
n
>
4
，化简得
n -3 = (-1)n-3 48 ，故只能是 n 为奇数且 n - 3 = 48 ．解得 n = 51．

2019年第十一届全国大学生数学竞赛初赛数学专业(B类)试题

2019年第十一届全国大学生数学竞赛数学专业竞赛（B 卷）试题一、（本题15分）设1L 和2L 是空间中的两条不垂直的异面直线，点B 是它们公垂线段的中点。

点1A 和2A 分别在1L 和2L 上滑动，使得12A B A B ⊥. 证明直线12A A 的轨迹是单叶双曲面。

二、(本题10分)计算()()220190d 11x x x +∞++⎰三、(本题15分)设数列{}n x 满足：()110,ln 1,1,2,n n x x x n +>=+= . 证明：{}n x 收敛并求其极限值. 四、(本题15分)设{}1,,n 是n 维实线性空间V 的一组基，令1210n n +++++=证明：（1）对{}11111,2,,1,,,,,,i i n i n -++=+ 都构成V 的基；（2）V α∀∈，在（1）中的1n +组基中，必存在一组基使α在此基下的坐标分量均非负；（3）若1122n n a a a α=+++ ，且(1,2,,)i a i n = 互不相同，则在（1）中的1n +组基中，满足（2）中非负坐标表示的基是唯一的.五、(本题20分)设A 是数域F 上的n 阶矩阵，若(2n n A I I =表示单位矩阵），则称A 为对合矩阵. 试证：（1）若A 是n 阶对合矩阵，则()()rank rank n n I A I A n ++-=；（2）n 阶对合矩阵A 一定可以对角化，其相似对角形为00r n r I I -⎛⎫ ⎪ ⎪ ⎪- ⎪⎝⎭，其中 ()rank n A r I =+；（3）若A ，B 均是n 阶对合矩阵，且AB BA =，则存在可逆矩阵P ，使得1P AP -和1P BP -同时为对角矩阵.六、(本题15分)设函数()f x 为闭区间,a b ⎡⎤⎢⎥⎣⎦上的连续凹函数，满足()()0,0f a f b =>且()f x 在x a =处存在非零的右导数. 对2n ≥，记()11:(),[,]n n n k k k k k S kx kf x f b x a b ==⎧⎫⎪⎪⎪⎪==∈⎨⎬⎪⎪⎪⎪⎩⎭∑∑（1）证明对(0,())f b α∀∈，存在唯一(,)x a b ∈使得()f x α=；（2）求()lim sup inf .n n n S S →∞-七、(本题10分)设正项级数11n n a ∞=∑收敛. 证明级数221n n nn a S ∞=∑收敛，其中1n k k n a S ==∑.。

2019年全国高中数学联合竞赛试题及解析(AB合版)

2019年全国高中数学联合竞赛一试（A 卷）参考答案及评分标准说明：1. 评阅试卷时，请依据本评分标准. 填空题只设8分和0分两档；其他各题的评阅，请严格按照本评分标准的评分档次给分，不得增加其他中间档次.2. 如果考生的解答方法和本解答不同，只要思路合理、步骤正确，在评卷时可参考本评分标准适当划分档次评分，解答题中第9小题4分为一个档次，第10、11小题5分为一个档次，不得增加其他中间档次．一、填空题：本大题共8小题，每小题8分，满分64分． 1. 已知正实数a 满足8(9)a a a a =，则log (3)a a 的值为．答案：916．解：由条件知189a a =，故9163a a ==，所以9log (3)16a a =．2. 若实数集合{1,2,3,}x 的最大元素与最小元素之差等于该集合的所有元素之和，则x 的值为．答案：32-．解：假如0x ³，则最大、最小元素之差不超过max{3,}x ，而所有元素之和大于max{3,}x ，不符合条件．故0x <，即x 为最小元素．于是36x x -=+，解得32x =-．3. 平面直角坐标系中，e 是单位向量，向量a 满足2a e⋅=，且25a a te£+对任意实数t 成立，则a的取值范围是．答案：．解：不妨设(1,0)e =．由于2a e ⋅=，可设(2,)a s=，则对任意实数t ，有2245s a a te +=£+= 这等价于245s s +£，解得[1,4]s Î，即2[1,16]s Î．于是a=Î．4. 设,A B 为椭圆G 的长轴顶点，,E F 为G 的两个焦点，4,AB =2AF =P 为G 上一点，满足2PE PF ⋅=，则PEF D 的面积为．答案：1．解：不妨设平面直角坐标系中G 的标准方程为22221(0)x y a b a b +=>>．根据条件得24,2a AB a AF ====可知2,1a b ==，且EF ==由椭圆定义知24PE PF a +==，结合2PE PF ⋅=得()2222212PE PF PE PF PE PF EF +=+-⋅==，所以EPF 为直角，进而112PEF S PE PF D =⋅⋅=．5.在1,2,3,,10 中随机选出一个数a ，在1,2,3,,10 ----中随机选出一个数b ，则2a b +被3整除的概率为．答案：37100．解：数组(,)a b 共有210100=种等概率的选法．考虑其中使2a b +被3整除的选法数N ．若a 被3整除，则b 也被3整除．此时,a b 各有3种选法，这样的(,)a b 有239=组．若a 不被3整除，则21(mod3)a º，从而1(mod3)b º-．此时a 有7种选法，b 有4种选法，这样的(,)a b 有7428´=组．因此92837N =+=．于是所求概率为37100．6.对任意闭区间I ，用I M 表示函数sin y x =在I 上的最大值．若正数a 满足[0,][,2]2a a a M M =，则a 的值为．答案：56p 或1312p ．解：假如02a p<£，则由正弦函数图像性质得[0,][,2]0sin a a a M a M <=£，与条件不符．因此2a p>，此时[0,]1a M =，故[,2]12a a M =．于是存在非负整数k ，使得51322266k a a k p p p p +£<£+， ①且①中两处“£”至少有一处取到等号．当0k =时，得56a p =或1326a p =．经检验，513,612a p p =均满足条件．当1k ³时，由于13522266k k p p p p æö÷ç+<+÷ç÷çèø，故不存在满足①的a ．综上，a 的值为56p 或1312p ．7.如图，正方体ABCD EFGH -的一个截面经过顶点,A C 及棱EF 上一点K ，且将正方体分成体积比为3:1的两部分，则EKKF 的值为．答案．解：记a 为截面所在平面．延长,AK BF 交于点P ，则P在a 上，故直线CP 是a 与平面BCGF 的交线．设CP 与FG 交于点L ，则四边形AKLC 为截面．因平面ABC 平行于平面KFL ，且,,AK BF CL 共点P ，故ABC KFL -为棱台．不妨设正方体棱长为1，则正方体体积为1，结合条件知棱台ABC KFL -的体积14V =．设PF h =，则1KF FL PF h AB BC PB h ===+．注意到,PB PF 分别是棱锥P ABC -与棱锥P KFL -的高，于是111466P ABC P KFL V V V AB BC PB KF FL PF --==-=⋅⋅-⋅⋅ 3221331(1)1616(1)h h h h h h æöæö++÷ç÷ç÷ç=+-=÷÷çç÷ç÷èø÷ç++èø．化简得231h =，故h =1EK AE KF PF h ===． 8. 将6个数2,0,1,9,20,19按任意次序排成一行，拼成一个8位数（首位不为0），则产生的不同的8位数的个数为．答案：498．解：将2,0,1,9,20,19的首位不为0的排列的全体记为A ．易知55!600A =´=（这里及以下，X 表示有限集X 的元素个数）．将A 中2的后一项是0，且1的后一项是9的排列的全体记为B ；A 中2的后一项是0，但1的后一项不是9的排列的全体记为C ；A 中1的后一项是9，但2的后一项不是0的排列的全体记为D ．易知4!B =，5!B C +=，44!B D +=´，即24,96,72B C D ===．由B 中排列产生的每个8位数，恰对应B 中的224´=个排列（这样的排列中，20可与“2,0”互换，19可与“1,9”互换）．类似地，由C 或D 中排列产生的每个8位数，恰对应C 或D 中的2个排列．因此满足条件的8位数的个数为\()42B C DA B C D +++3600184836498422B C DA =---=---=．二、解答题：本大题共3小题，满分56分．解答应写出文字说明、证明过程或演算步骤．9.（本题满分16分）在ABC D 中，,,BC a CA b AB c ===．若b 是a 与c 的等比中项，且sin A 是sin()B A -与sin C 的等差中项，求cos B 的值．解：因b 是,a c 的等比中项，故存在0q >，满足2,b qa c q a ==． ①因sin A 是sin(),sin B A C -的等差中项，故2sin sin()sin sin()sin()2sin cos A B A C B A B A B A =-+=-++=．…………………4分结合正、余弦定理，得222sin cos sin 2a A b c a A b B bc+-===，即2222b c a ac +-=． …………………8分αLD F B K将①代入并化简，可知24212q q q +-=，即421q q =+，所以212q =． …………………12分进而2224222111cos 222c a b q q B ac q q +-+-====． …………………16分10. （本题满分20分）在平面直角坐标系xOy 中，圆W 与抛物线2:4y x G =恰有一个公共点，且圆W 与x 轴相切于G 的焦点F ．求圆W 的半径．解：易知G 的焦点F 的坐标为(1,0)．设圆W 的半径为(0)r r >．由对称性，不妨设W 在x 轴上方与x 轴相切于F ，故W 的方程为222(1)()x y r r -+-=． ①将24yx =代入①并化简，得2221204y y ry æö÷ç÷-+-=ç÷÷çèø．显然0y >，故 222221(4)12432y y r y y y æöæö÷+ç÷ç÷ç÷=-+=÷çç÷÷ç÷ç÷èøçèø． ② …………………5分根据条件，②恰有一个正数解y ，该y 值对应W 与G 的唯一公共点．考虑22(4)()(0)32y f y y y+=>的最小值．由平均值不等式知2244444333y y +=+++³，从而1()329f y y ³⋅=．当且仅当243y =，即3y =时，()f y取到最小值9． ………………15分由②有解可知9r ³．又假如9r >，因()f y 随y 连续变化，且0y +及y +¥时()f y 均可任意大，故②在0,3æççççèø及3æö÷ç÷+¥ç÷ç÷çèø上均有解，与解的唯一性矛盾．综上，仅有9r =满足条件（此时1,33æ÷ç÷ç÷ç÷çèø是W 与G 的唯一公共点）． …………………20分11. （本题满分20分）称一个复数数列{}n z 为“有趣的”，若11z =，且对任意正整数n ，均有2211420n n n n z z z z ++++=．求最大的常数C ，使得对一切有趣的数列{}n z 及任意正整数m ，均有12m z z z C +++³．解：考虑有趣的复数数列{}n z ．归纳地可知*0()n z n N ¹Î．由条件得2*114210()n n n nz z n z z N ++æöæö÷÷çç÷÷++=Îçç÷÷ç÷÷çèøèø，解得*11()4N n n z n z +-=Î．因此1112n n n n z z z z ++===，故 *11111()22N n n n z z n --=⋅=Î．①…………………5分进而有*11111()22N n n n n n n nz z z z n z ++-+=⋅+==Î． ②记*12()N m m T z z z m =+++Î．当*2()N m s s =Î时，利用②可得122122sm k k k T z z z z -=³+-+å21222k k k z z ¥-=>-+å212223k k ¥-==-=å． …………………10分当*21()N m s s =+Î时，由①、②可知21212221211112322s k k s s k k s k s z z z ¥¥+---=+=+=<==+⋅åå，故12212212s m k k s k T z z z z z -+=æö÷ç³+-+-÷ç÷çèøå212223k k k z z ¥-=>-+=å．当1m =时，1113T z ==>．以上表明3C =满足要求． …………………15分另一方面，当*1221221111,,()22N k k k k z z z k ++--===Î时，易验证知{}n z 为有趣的数列．此时2112211lim lim ()ss k k s s k T z z z ++ ¥¥==++å134lim 11833ss k ¥=-=+=+⋅=，这表明C不能大于3．综上，所求的C为3． …………………20分2019年全国高中数学联合竞赛加试（A 卷）参考答案及评分标准说明：1．评阅试卷时，请严格按照本评分标准的评分档次给分．2．如果考生的解答方法和本解答不同，只要思路合理、步骤正确，在评卷时可参考本评分标准适当划分档次评分，10分为一个档次，不得增加其他中间档次．一、（本题满分40分）如图，在锐角ABC D 中，M 是BC 边的中点．点P 在ABC D 内，使得AP 平分BAC ．直线MP 与,ABP ACP D D 的外接圆分别相交于不同于点P 的两点,D E ．证明：若DE MP =，则2BC BP =．证明：延长PM 到点F ，使得MF ME =．连接,,BF BD CE ．由条件可知BDP BAP CAP CEP CEM = = = = ． ………………10分因为BM CM =且EM FM =，所以BF CE =且//BF CE ．于是F CEM BDP = = ，进而BD BF =． ………………20分又DE MP =，故DP EM FM ==．于是在等腰BDF D 中，由对称性得BP BM =．从而22BC BM BP ==． ………………40分二、（本题满分40分）设整数122019,,,a a a 满足122019199a a a =£££=．记22212201913243520172019()()f a a a a a a a a a a a =+++-++++．求f 的最小值0f ．并确定使0f f =成立的数组122019(,,,)a a a 的个数．解：由条件知2017222221220182019212()i i i f a a aaa a +==++++-å．①由于12,a a 及2(1,2,,2016)i i a a i +-=均为非负整数，故有221122,a a a a ³³，且222()(1,2,,2016)i i i i a a a a i ++-³-=．于是201620162221221222017201811()()i i i i i i a a a a a a a a a a ++==++-³++-=+åå．②………………10分由①、②得2222017201820192017201820192()f a a a a a a ³++-++，结合201999a =及201820170a a ³>，可知()22220172017201712(99)992f a a a ³+-++22017(49)74007400a =-+³．③………………20分另一方面，令1219201920211920220191,(1,2,,49),99k k a a a a a k k a +-+========，此时验证知上述所有不等式均取到等号，从而f 的最小值07400f =．………………30分以下考虑③的取等条件．此时2017201849a a ==，且②中的不等式均取等，即121a a ==，2{0,1}(1,2,,2016)i i a a i +-Î=．因此122018149a a a =£££=，且对每个(149)k k ££，122018,,,a a a 中至少有两项等于k ．易验证知这也是③取等的充分条件．对每个(149)k k ££，设122018,,,a a a 中等于k 的项数为1k n +，则k n 为正整数，且1249(1)(1)(1)2018n n n ++++++=，即12491969n n n +++=．该方程的正整数解1249(,,,)n n n 的组数为481968C ，且每组解唯一对应一个使④取等的数组122019(,,,)a a a ，故使0f f =成立的数组122019(,,,)a a a 有481968C 个．………………40分三、（本题满分50分）设m 为整数，2m ||³．整数数列12,,a a 满足：12,a a 不全为零，且对任意正整数n ，均有21n n n a a ma ++=-．证明：若存在整数,r s (2)r s >³使得1r s a a a ==，则r s m ||-³．证明：不妨设12,a a 互素（否则，若12(,)1a a d =>，则1a d 与2ad互素，并且用123,,,a a a d d d代替123,,,a a a ，条件与结论均不改变）．由数列递推关系知234(mod )a a a m || ººº．① 以下证明：对任意整数3n ³，有2212((3))(mod )n a a a n a m m º-+-．②………………10分事实上，当3n =时②显然成立．假设n k =时②成立（其中k 为某个大于2的整数），注意到①，有212(mod )k ma ma m -º，结合归纳假设知112122((3))k k k a a ma a a k a m ma +-=-º-+--2212((2))(mod )a a k a m º-+-，即1n k =+时②也成立．因此②对任意整数3n ³均成立． ………………20分注意，当12a a =时，②对2n =也成立．设整数,(2)r s r s >³，满足1r s a a a ==．若12a a =，由②对2n ³均成立，可知2212212((3))((3))(mod )r s a a r a m a a a a s a m m -+-º=º-+-，即1212(3)(3)(mod )a r a a s a m ||+-º+-，即2()0(mod )r s a m ||-º．③若12a a ¹，则12r s a a a a ==¹，故3r s >³．此时由于②对3n ³均成立，故类似可知③仍成立． ………………30分我们证明2,a m 互素．事实上，假如2a 与m 存在一个公共素因子p ，则由①得p 为234,,,a a a 的公因子，而12,a a 互素，故p 1a ，这与1r s a a a ==矛盾．因此，由③得0(mod )r s m ||-º．又r s >，所以r s m ||-³．………………50分四、（本题满分50分）设V 是空间中2019个点构成的集合，其中任意四点不共面．某些点之间连有线段，记E 为这些线段构成的集合．试求最小的正整数n ，满足条件：若E 至少有n 个元素，则E 一定含有908个二元子集，其中每个二元子集中的两条线段有公共端点，且任意两个二元子集的交为空集．解：为了叙述方便，称一个图中的两条相邻的边构成一个“角”．先证明一个引理：设(,)G V E =是一个简单图，且G 是连通的，则G 含有||2E ⎡⎤⎢⎥⎣⎦个两两无公共边的角（这里[]a 表示实数a 的整数部分）．引理的证明：对E 的元素个数E 归纳证明．当0,1,2,3E =时，结论显然成立．下面假设4E ≥，并且结论在E 较小时均成立．只需证明，在G 中可以选取两条边,a b 构成一个角，在G 中删去,a b 这两条边后，剩下的图含有一个连通分支包含||2E -条边．对这个连通分支应用归纳假设即得结论成立．考虑G 中的最长路12:k P v v v ，其中21,,,k v v v 是互不相同的顶点．因为G 连通，故3k ≥．情形1：1deg()2v ≥．由于P 是最长路，1v 的邻点均在2,,k v v 中，设1i v v E ∈，其中3i k ≤≤．则121{,}i v v v v 是一个角，在E 中删去这两条边．若1v 处还有第三条边，则剩下的图是连通的；若1v 处仅有被删去的两条边，则1v 成为孤立点，其余顶点仍互相连通．总之在剩下的图中有一个连通分支含有2E -条边．情形2：1deg()1v =，2deg()2v =．则1223{,}v v v v 是一个角，在G 中删去这两条边后，12,v v 都成为孤立点，其余的点互相连通，因此有一个连通分支含有2E -条边．情形3：1deg()1v =，2deg()3v ≥，且2v 与4,,k v v 中某个点相邻．则1223{,}v v v v是一个角，在G 中删去这两条边后，1v 成为孤立点，其余点互相连通，因此有一个连通分支含有2E -条边．情形4：1deg()1v =，2deg()3v ≥，且2v 与某个13{,,,}k u v v v ∈/ 相邻．由于P 是最长路，故u 的邻点均在2,,k v v 之中．因122{,}v v v u 是一个角，在G 中删去这两条边，则1v 是孤立点．若u 处仅有边2uv ，则删去所述边后u 也是孤立点，而其余点互相连通．若u 处还有其他边i uv ，3i k ≤≤，则删去所述边后，除1v 外其余点互相连通．总之，剩下的图中有一个连通分支含有2E -条边．引理获证． ………………20分回到原题，题中的V 和E 可看作一个图(,)G V E =．首先证明2795n ≥．设122019{,,,}V v v v = ．在1261,,,v v v 中，首先两两连边，再删去其中15条边（例如1311216,,,v v v v v v ），共连了26115C 1815-=条边，则这61个点构成的图是连通图．再将剩余的2019611958-=个点配成979对，每对两点之间连一条边，则图G 中一共连了181********+=条线段．由上述构造可见，G 中的任何一个角必须使用1261,,,v v v 相连的边，因此至多有18159072⎡⎤⎢=⎥⎣⎦个两两无公共边的角．故满足要求的n 不小于2795． ………………30分另一方面，若2795E ≥，可任意删去若干条边，只考虑2795E =的情形．设G 有k 个连通分支，分别有1,,k m m 个点，及1,,k e e 条边．下面证明1,,k e e 中至多有979个奇数．反证法，假设1,,k e e 中有至少980个奇数，由于12795k e e ++= 是奇数，故1,,k e e 中至少有981个奇数，故981k ≥．不妨设12981,,,e e e 都是奇数，显然12981,,,2m m m ≥ ．令9812k m m m =++≥ ，则有2C 1980)(i m i e i ≥≤≤，2981C m k e e ≥++ ，故98022112795C C imk i i i m e ===≤+∑∑． ① 利用组合数的凸性，即对3x y ≥≥，有222211C C C C x y x y +-+≤+，可知当1980,,,m m m 由980个2以及一个59构成时，980221C C imm i =+∑取得最大值．于是 98022225921C C C 980C 26912795imm i =≤=<++∑，这与①矛盾．从而1,,k e e 中至多有979个奇数． ………………40分对每个连通分支应用引理，可知G 中含有N 个两两无公共边的角，其中1111979(2795979)908222kki i i i e N e ==⎛⎫⎡⎤=≥-=-= ⎪⎢⎥⎣⎦⎝⎭∑∑．综上，所求最小的n 是2795． ………………50分2019年全国高中数学联合竞赛一试（B 卷）参考答案及评分标准说明：1. 评阅试卷时，请依据本评分标准. 填空题只设8分和0分两档；其他各题的评阅，请严格按照本评分标准的评分档次给分，不得增加其他中间档次.2. 如果考生的解答方法和本解答不同，只要思路合理、步骤正确，在评卷时可参考本评分标准适当划分档次评分，解答题中第9小题4分为一个档次，第10、11小题5分为一个档次，不得增加其他中间档次．一、填空题：本大题共8小题，每小题8分，满分64分．1. 已知实数集合{1,2,3,}x 的最大元素等于该集合的所有元素之和，则x 的值为．答案：3-．解：条件等价于1,2,3,x 中除最大数以外的另三个数之和为0．显然0x <，从而120x ++=，得3x =-．2. 若平面向量(2,1)m a =-与1(21,2)m m b +=-垂直，其中m 为实数，则a 的模为．答案解：令2m t =，则0t >．条件等价于(1)(1)20t t t ⋅-+-⋅=，解得3t =．因此a=．3. 设,(0,)a b p Î，cos ,cos a b 是方程25310x x --=的两根，则sin sin a b 的值为．答案：5．解：由条件知31cos cos ,cos cos 55a b a b +==-，从而222(sin sin )(1cos )(1cos )a b a b =--22221cos cos cos cos a b a b=--+2222437(1cos cos )(cos cos )5525a b a b æöæö÷çç=+-+=-=÷çç÷ççèøè．又由,(0,)a b p Î知sin sin 0a b >，从而sin sin 5a b =． 4. 设三棱锥P ABC -满足3,2PA PB AB BC CA =====，则该三棱锥的体积的最大值为．答案：3．解：设三棱锥P ABC -的高为h ．取M 为棱AB 的中点，则h PM £==．当平面PAB 垂直于平面ABC 时，h 取到最大值．此时三棱锥P ABC -的体积取到最大值11333ABC S D ⋅==．5. 将5个数2,0,1,9,2019按任意次序排成一行，拼成一个8位数（首位不为0），则产生的不同的8位数的个数为．答案：95．解：易知2,0,1,9,2019的所有不以0为开头的排列共有44!96´=个．其中，除了(2,0,1,9,2019)和(2019,2,0,1,9)这两种排列对应同一个数20192019，其余的数互不相等．因此满足条件的8位数的个数为96195-=．6. 设整数4n >，(1)n x +的展开式中4n x -与xy 两项的系数相等，则n 的值为．答案：51．解：注意到0(1)C 1)nnr n r rnr x x -=+=å．其中4n x -项仅出现在求和指标4r =时的展开式444C 1)n n x -中，其4n x -项系数为44(1)(2)(3)(1)C 24nn n n n ----=．而xy 项仅出现在求和指标1r n =-时的展开式11C 1)n n nx --⋅中，其xy 项系数为12331C C 4(1)(1)2(1)(2)n n n n n n n n ----⋅-=---．因此有3(1)(2)(3)(1)2(1)(2)24n n n n n n n n ----=---．注意到4n >，化简得33(1)48n n --=-，故只能是n 为奇数且348n -=．解得51n =．7. 在平面直角坐标系中，若以(1,0)r +为圆心、r 为半径的圆上存在一点(,)a b 满足24b a ³，则r 的最小值为．答案：4．解：由条件知222(1)a r b r --+=，故22224(1)2(1)(1)a b r a r r a a £=---=---．即22(1)210a r a r --++£．上述关于a 的一元二次不等式有解，故判别式2(2(1))4(21)4(4)0r r r r --+=-³，解得4r ³．经检验，当4r =时，(,)(3,a b =满足条件．因此r 的最小值为4． 8. 设等差数列{}n a 的各项均为整数，首项12019a =，且对任意正整数n ，总存在正整数m ，使得12n m a a a a +++=．这样的数列{}n a 的个数为．答案：5．解：设{}n a 的公差为d ．由条件知12k a a a +=（k 是某个正整数），则 112(1)a d a k d +=+-，即1(2)k d a -=，因此必有2k ¹，且12ad k =-．这样就有1111(1)2n n a a n d a a k -=+-=+-，而此时对任意正整数n ，12111(1)(1)(1)22n n n n n a a a a n d a n a d --+++=+=+-+ 1(1)(1)(2)2n n a n k d æö-÷ç=+--+÷ç÷çèø，确实为{}n a 中的一项．因此，仅需考虑使12|k a -成立的正整数k 的个数．注意到2019为两个素数3与673之积，易知2k -可取1,1,3,673,2019-这5个值，对应得到5个满足条件的等差数列．二、解答题：本大题共3小题，满分56分．解答应写出文字说明、证明过程或演算步骤．9.（本题满分16分）在椭圆G 中，F 为一个焦点，,A B 为两个顶点．若3,2FA FB ==，求AB 的所有可能值．解：不妨设平面直角坐标系中椭圆G 的标准方程为22221(0)x y a b a b +=>>，并记c =F 为G 的右焦点．易知F 到G 的左顶点的距离为a c +，到右顶点的距离为a c -，到上、下顶点的距离均为a ．分以下情况讨论：(1) ,A B 分别为左、右顶点．此时3,2a c a c +=-=，故25AB a ==（相应地，2()()6b a c a c =+-=，G 的方程为2241256x y +=）． …………………4分 (2) A 为左顶点，B 为上顶点或下顶点．此时3,2a c a +==，故1c =，进而2223b a c =-=，所以AB ==G 的方程为22143x y +=）．…………………8分 (3) A 为上顶点或下顶点，B 为右顶点．此时3,2a a c =-=，故1c =，进而2228b a c =-=，所以AB ==G 的方程为22198x y +=）．…………………12分综上可知，AB的所有可能值为5,． …………………16分10. （本题满分20分）设,,a b c 均大于1，满足lg log 3,lg log 4.b a a c b c ì+=ïïíï+=ïî求lg lg a c ⋅的最大值．解：设lg ,lg ,lg a x b y c z ===，由,,1a b c >可知,,0x y z >．由条件及换底公式知3,4z zx y y x+=+=，即34xy z y x +==．…………………5分由此，令3,4(0)x t y t t ==>，则241212z x xy t t =-=-．其中由0z >可知(0,1)t Î． …………………10分因此，结合三元平均值不等式得2lg lg 312(1)18(22)a c xz t t t t t ==⋅-=⋅-33(22)2161818333t t t æöæö++-÷çç£⋅=⋅=÷çç÷ççèèø．当22t t =-，即23t =（相应的,,a b c 分别为8833100,10,10）时，lg lg a c 取到最大值163． …………………20分11. （本题满分20分）设复数数列{}n z 满足：11z =，且对任意正整数n ，均有2211420n n n n z z z z ++++=．证明：对任意正整数m ，均有123m z z z +++<．证明：归纳地可知*0()n z n N ¹Î．由条件得2*114210()n n n n z z n z z N ++æöæö÷çç÷++=Îçç÷çç÷èøèø，解得*11()4N n n z n z +-=Î． …………………5分因此1112n n nnz z z z ++===，故*11111()22N n n n z z n --=⋅=Î． ①进而有*11111()22N n n n n n n nz z z z n z ++-+=⋅+==Î． ②…………………10分当m 为偶数时，设*2()N m s s =Î．利用②可得122122122111123sm k k k k k k k k z z z z z z z ¥¥---===+++£+<+==ååå． …………………15分当m 为奇数时，设21()N m s s =+Î．由①、②可知21212221211112322s k k s s k k s k s z z z ¥¥+---=+=+=<==+⋅åå，故1221221212113s m k k s k k k k z z z z z z z z ¥-+-==æö÷ç+++£++<+=÷ç÷çèøåå．综上，结论获证． …………………20分2019年全国高中数学联合竞赛加试（B 卷）参考答案及评分标准说明：1．评阅试卷时，请严格按照本评分标准的评分档次给分．2．如果考生的解答方法和本解答不同，只要思路合理、步骤正确，在评卷时可参考本评分标准适当划分档次评分，10分为一个档次，不得增加其他中间档次．一、（本题满分40分）设正实数12100,,,a a a 满足101(1,2,,50)i i a a i -³=．记112(1,2,,99)k k kka x k a a a +==+++．证明：29912991x x x £．证明：注意到12100,,,0a a a >．对1,2,,99k =，由平均值不等式知121210kk k k a a a a a a æöç<£çç+++èø， ……………10分从而有9999299112991111212kk k k k k k k ka k x x x a a a a a a a ++==æö÷ç÷=£ç÷÷ç+++èø ． ①………………20分记①的右端为T ，则对任意1,2,,100i =，i a 在T 的分子中的次数为1i -，在T 的分母中的次数为100i -．从而10121005050210121012(101)101101101111ii i i i i i ii i i ia T a a a a -------===æö÷ç÷===ç÷ç÷èø ．………………30分又1010(1,2,,50)i i a a i -<£=，故1T £，结合①得29912991x x x T ££． ………………40分二、（本题满分40分）求满足以下条件的所有正整数n ：(1) n 至少有4个正约数；(2) 若12k d d d <<< 是n 的所有正约数，则21321,,,k k d d d d d d ---- 构成等比数列．解：由条件可知4k ≥，且3212112kk k k d d d d d d d d -----=--． ………………10分易知112231,,,k k k n nd d n d d d d --====，代入上式得3222231n n d d d n n d d d --=--，化简得223223()(1)d d d d -=-． ………………20分由此可知3d 是完全平方数．由于2d p =是n 的最小素因子，3d 是平方数，故只能23d p =． ………………30分从而序列21321,,,k k d d d d d d ---- 为23212,1,,,k k p p p p p p p ------ ，即123,,,,k d d d d 为21,1,,,k p p p - ，而此时相应的n 为1k p -．综上可知，满足条件的n 为所有形如a p 的数，其中p 是素数，整数3a ≥． ………………40分三、（本题满分50分）如图，点,,,,A B C D E在一条直线上顺次排列，满足BC CD ==，点P 在该直线外，满足PB PD =．点,K L 分别在线段,PB PD 上，满足KC 平分BKE ，LC 平分ALD ．证明：,,,A K L E 四点共圆．（答题时请将图画在答卷纸上）证明：令1,(0)AB BC CD t ===>，由条件知2DE t =．注意到180BKE ABK PDE DEK < = < - ，可在CB 延长线上取一点A ¢，使得A KE ABK A BK ¢¢ = = ． ………………10分此时有A BK A KE ∽¢¢D D ，故A B A K BKA K A E KE¢¢==¢¢． ………………20分又KC 平分BKE ，故211BK BC t KE CE t t t===++．于是有 22112A B A B A K BK AB A E A K A E KE t t AEæö¢¢¢÷ç=⋅===÷ç÷ç¢¢¢èø++． …………30分由上式两端减1，得BE BEA E AE=¢，从而A A ¢=．因此AKE A KE ABK ¢ = = ．同理可得ALE EDL = ．而ABK EDL = ，所以AKE ALE = ．因此,,,A K L E 四点共圆． ………………50分四、（本题满分50分）将一个凸2019边形的每条边任意染为红、黄、蓝三种颜色之一，每种颜色的边各673条．证明：可作这个凸2019边形的2016条在内部互不相交的对角线将其剖分成2017个三角形，并将所作的每条对角线也染AA (为红、黄、蓝三种颜色之一，使得每个三角形的三条边或者颜色全部相同，或者颜色互不相同．证明：我们对5n ≥归纳证明加强的命题：如果将凸n 边形的边染为三种颜色,,a b c ，并且三种颜色的边均至少有一条，那么可作满足要求的三角形剖分． ………………10分当5n =时，若三种颜色的边数为1，1，3，由对称性，只需考虑如下两种情形，分别可作图中所示的三角形剖分．若三种颜色的边数为1，2，2，由对称性，只需考虑如下三种情形，分别可作图中所示的三角形剖分．………………20分假设结论对(5)n n ≥成立，考虑1n +的情形，将凸1n +边形记为121n A A A + ．情形1：有两种颜色的边各只有一条．不妨设,a b 色边各只有一条．由于16n +≥，故存在连续两条边均为c 色，不妨设是111,n n n A A A A ++．作对角线1n A A ，并将1n A A 染为c 色，则三角形11n n A A A +的三边全部同色．此时凸n 边形12n A A A 的三种颜色的边均至少有一条，由归纳假设，可对其作符合要求的三角形剖分．………………30分情形2：某种颜色的边只有一条，其余颜色的边均至少两条．不妨设a 色边只有一条，于是可以选择两条相邻边均不是a 色，不妨设111,n n n A A A A ++均不是a 色，作对角线1n A A ，则1n A A 有唯一的染色方式，使得三角形11n n A A A +的三边全部同色或互不同色．此时凸n 边形12n A A A 的三种颜色的边均至少有一条，由归纳假设，可对其作符合要求的三角形剖分． ………………40分情形3：每种颜色的边均至少两条．作对角线1n A A ，则1n A A 有唯一的染色方式，使得三角形11n n A A A +的三边全部同色或互不同色．此时凸n 边形12n A A A 的三种颜色的边均至少有一条，由归纳假设，可对其作符合要求的三角形剖分．综合以上3种情形，可知1n +的情形下结论也成立．由数学归纳法，结论获证． ………………50分。

2019年全国高中数学联赛A+B卷(含答案)

2019年全国高中数学联合竞赛一试（A 卷）参考答案及评分标准说明：1. 评阅试卷时，请依据本评分标准. 填空题只设8分和0分两档；其他各题的评阅，请严格按照本评分标准的评分档次给分，不得增加其他中间档次.2. 如果考生的解答方法和本解答不同，只要思路合理、步骤正确，在评卷时可参考本评分标准适当划分档次评分，解答题中第9小题4分为一个档次，第10、11小题5分为一个档次，不得增加其他中间档次．一、填空题：本大题共8小题，每小题8分，满分64分． 1. 已知正实数a 满足8(9)a a a a =，则log (3)a a 的值为．答案：916．解：由条件知189a a =，故9163a a ==，所以9log (3)16a a =．2. 若实数集合{1,2,3,}x 的最大元素与最小元素之差等于该集合的所有元素之和，则x 的值为．答案：32-．解：假如0x ³，则最大、最小元素之差不超过max{3,}x ，而所有元素之和大于max{3,}x ，不符合条件．故0x <，即x 为最小元素．于是36x x -=+，解得32x =-．3. 平面直角坐标系中，e 是单位向量，向量a 满足2a e⋅=，且25a a te£+对任意实数t 成立，则a的取值范围是．答案：．解：不妨设(1,0)e =．由于2a e ⋅=，可设(2,)a s=，则对任意实数t ，有2245s a a te +=£+= 这等价于245s s +£，解得[1,4]s Î，即2[1,16]s Î．于是a=Î．4. 设,A B 为椭圆G 的长轴顶点，,E F 为G 的两个焦点，4,AB =2AF =P 为G 上一点，满足2PE PF ⋅=，则PEF D 的面积为．答案：1．解：不妨设平面直角坐标系中G 的标准方程为22221(0)x y a b a b +=>>．根据条件得24,2a AB a AF ====可知2,1a b ==，且EF ==由椭圆定义知24PE PF a +==，结合2PE PF ⋅=得()2222212PE PF PE PF PE PF EF +=+-⋅==，所以EPF 为直角，进而112PEF S PE PF D =⋅⋅=．5. 在1,2,3,,10 中随机选出一个数a ，在1,2,3,,10 ----中随机选出一个数b ，则2a b +被3整除的概率为．答案：37100．解：数组(,)a b 共有210100=种等概率的选法．考虑其中使2a b +被3整除的选法数N ．若a 被3整除，则b 也被3整除．此时,a b 各有3种选法，这样的(,)a b 有239=组．若a 不被3整除，则21(mod3)a º，从而1(mod3)b º-．此时a 有7种选法，b 有4种选法，这样的(,)a b 有7428´=组．因此92837N =+=．于是所求概率为37100．6. 对任意闭区间I ，用I M 表示函数sin y x =在I 上的最大值．若正数a 满足[0,][,2]2a a a M M =，则a 的值为．答案：56p 或1312p ．解：假如02a p<£，则由正弦函数图像性质得[0,][,2]0sin a a a M a M <=£，与条件不符．因此2a p >，此时[0,]1a M =，故[,2]12a a M =．于是存在非负整数k ，使得51322266k a a k p p p p +£<£+， ①且①中两处“£”至少有一处取到等号．当0k =时，得56a p =或1326a p =．经检验，513,612a p p =均满足条件．当1k ³时，由于13522266k k p p p p æö÷ç+<+÷ç÷çèø，故不存在满足①的a ．综上，a 的值为56p 或1312p ．7. 如图，正方体ABCD EFGH -的一个截面经过顶点,A C 及棱EF 上一点K ，且将正方体分成体积比为3:1的两部分，则EKKF 的值为．答案．解：记a 为截面所在平面．延长,AK BF 交于点P ，则P在a 上，故直线CP 是a 与平面BCGF 的交线．设CP 与FG 交于点L ，则四边形AKLC 为截面．因平面ABC 平行于平面KFL ，且,,AK BF CL 共点P ，故ABC KFL -为棱台．不妨设正方体棱长为1，则正方体体积为1，结合条件知棱台ABC KFL -的体积14V =．设PF h =，则1KF FL PF h AB BC PB h ===+．注意到,PB PF 分别是棱锥P ABC -与棱锥P KFL -的高，于是111466P ABC P KFL V V V AB BC PB KF FL PF --==-=⋅⋅-⋅⋅ 3221331(1)1616(1)h h h h h h æöæö++÷ç÷ç÷ç=+-=÷÷çç÷ç÷èø÷ç++èø．化简得231h =，故h =1EK AE KF PF h ===． 8. 将6个数2,0,1,9,20,19按任意次序排成一行，拼成一个8位数（首位不为0），则产生的不同的8位数的个数为．答案：498．解：将2,0,1,9,20,19的首位不为0的排列的全体记为A ．易知55!600A =´=（这里及以下，X 表示有限集X 的元素个数）．将A 中2的后一项是0，且1的后一项是9的排列的全体记为B ；A 中2的后一项是0，但1的后一项不是9的排列的全体记为C ；A 中1的后一项是9，但2的后一项不是0的排列的全体记为D ．易知4!B =，5!B C +=，44!B D +=´，即24,96,72B C D ===．由B 中排列产生的每个8位数，恰对应B 中的224´=个排列（这样的排列中，20可与“2,0”互换，19可与“1,9”互换）．类似地，由C 或D 中排列产生的每个8位数，恰对应C 或D 中的2个排列．因此满足条件的8位数的个数为\()42B C DA B C D +++3600184836498422B C DA =---=---=．二、解答题：本大题共3小题，满分56分．解答应写出文字说明、证明过程或演算步骤．9.（本题满分16分）在ABC D 中，,,BC a CA b AB c ===．若b 是a 与c 的等比中项，且sin A 是sin()B A -与sin C 的等差中项，求cos B 的值．解：因b 是,a c 的等比中项，故存在0q >，满足2,b qa c q a ==． ①因sin A 是sin(),sin B A C -的等差中项，故2sin sin()sin sin()sin()2sin cos A B A C B A B A B A =-+=-++=．…………………4分结合正、余弦定理，得222sin cos sin 2a A b c a A b B bc+-===，即2222b c a ac +-=． …………………8分αLD F B K将①代入并化简，可知24212q q q +-=，即421q q =+，所以212q =． …………………12分进而2224222111cos 222c a b q q B ac q q +-+-====． …………………16分10. （本题满分20分）在平面直角坐标系xOy 中，圆W 与抛物线2:4y x G =恰有一个公共点，且圆W 与x 轴相切于G 的焦点F ．求圆W 的半径．解：易知G 的焦点F 的坐标为(1,0)．设圆W 的半径为(0)r r >．由对称性，不妨设W 在x 轴上方与x 轴相切于F ，故W 的方程为222(1)()x y r r -+-=． ①将24yx =代入①并化简，得2221204y y ry æö÷ç÷-+-=ç÷÷çèø．显然0y >，故 222221(4)12432y y r y y y æöæö÷+ç÷ç÷ç÷=-+=÷çç÷÷ç÷ç÷èøçèø． ② …………………5分根据条件，②恰有一个正数解y ，该y 值对应W 与G 的唯一公共点．考虑22(4)()(0)32y f y y y+=>的最小值．由平均值不等式知2244444333y y +=+++³，从而1()329f y y ³⋅=．当且仅当243y =，即3y =时，()f y取到最小值9． ………………15分由②有解可知9r ³．又假如9r >，因()f y 随y 连续变化，且0y +及y +¥时()f y 均可任意大，故②在0,3æççççèø及3æö÷ç÷+¥ç÷ç÷çèø上均有解，与解的唯一性矛盾．综上，仅有9r =满足条件（此时1,33æ÷ç÷ç÷ç÷çèø是W 与G 的唯一公共点）． …………………20分11. （本题满分20分）称一个复数数列{}n z 为“有趣的”，若11z =，且对任意正整数n ，均有2211420n n n n z z z z ++++=．求最大的常数C ，使得对一切有趣的数列{}n z 及任意正整数m ，均有12m z z z C +++³．解：考虑有趣的复数数列{}n z ．归纳地可知*0()n z n N ¹Î．由条件得2*114210()n n n nz z n z z N ++æöæö÷÷çç÷÷++=Îçç÷÷ç÷÷çèøèø，解得*11()4N n n z n z +-=Î．因此1112n n n n z z z z ++===，故 *11111()22N n n n z z n --=⋅=Î．①…………………5分进而有*11111()22N n n n n n n n z z z z n z ++-+=⋅+==Î． ②记*12()N m m T z z z m =+++Î．当*2()N m s s =Î时，利用②可得122122sm k k k T z z z z -=³+-+å21222k k k z z ¥-=>-+å212223k k ¥-==-=å．…………………10分当*21()N m s s =+Î时，由①、②可知21212221211112322s k k s s k k s k s z z z ¥¥+---=+=+=<==+⋅åå，故12212212s m k k s k T z z z z z -+=æö÷ç³+-+-÷ç÷çèøå212223k k k z z ¥-=>-+=å．当1m =时，1113T z ==>．以上表明3C =满足要求． …………………15分另一方面，当*1221221111,,()22N k k k k z z z k ++--===Î时，易验证知{}n z 为有趣的数列．此时2112211lim lim ()ss k k s s k T z z z ++ ¥¥==++å134lim 11833ss k ¥=-=+=+⋅=，这表明C不能大于3．综上，所求的C为3． …………………20分2019年全国高中数学联合竞赛一试（B 卷）参考答案及评分标准说明：1. 评阅试卷时，请依据本评分标准. 填空题只设8分和0分两档；其他各题的评阅，请严格按照本评分标准的评分档次给分，不得增加其他中间档次.2. 如果考生的解答方法和本解答不同，只要思路合理、步骤正确，在评卷时可参考本评分标准适当划分档次评分，解答题中第9小题4分为一个档次，第10、11小题5分为一个档次，不得增加其他中间档次．一、填空题：本大题共8小题，每小题8分，满分64分．1. 已知实数集合{1,2,3,}x 的最大元素等于该集合的所有元素之和，则x 的值为．答案：3-．解：条件等价于1,2,3,x 中除最大数以外的另三个数之和为0．显然0x <，从而120x ++=，得3x =-．2. 若平面向量(2,1)m a =-与1(21,2)m m b +=-垂直，其中m 为实数，则a 的模为．答案解：令2m t =，则0t >．条件等价于(1)(1)20t t t ⋅-+-⋅=，解得3t =．因此a=．3. 设,(0,)a b p Î，cos ,cos a b 是方程25310x x --=的两根，则sin sin a b 的值为．答案：5．解：由条件知31cos cos ,cos cos 55a b a b +==-，从而222(sin sin )(1cos )(1cos )a b a b =--22221cos cos cos cos a b a b=--+2222437(1cos cos )(cos cos )5525a b a b æöæö÷çç=+-+=-=÷çç÷ççèøè．又由,(0,)a b p Î知sin sin 0a b >，从而sin sin 5a b =． 4. 设三棱锥P ABC -满足3,2PA PB AB BC CA =====，则该三棱锥的体积的最大值为．答案：3．解：设三棱锥P ABC -的高为h ．取M 为棱AB 的中点，则h PM £==．当平面PAB 垂直于平面ABC 时，h 取到最大值．此时三棱锥P ABC -的体积取到最大值11333ABC S D ⋅==．5. 将5个数2,0,1,9,2019按任意次序排成一行，拼成一个8位数（首位不为0），则产生的不同的8位数的个数为．答案：95．解：易知2,0,1,9,2019的所有不以0为开头的排列共有44!96´=个．其中，除了(2,0,1,9,2019)和(2019,2,0,1,9)这两种排列对应同一个数20192019，其余的数互不相等．因此满足条件的8位数的个数为96195-=．6. 设整数4n >，(1)n x +的展开式中4n x -与xy 两项的系数相等，则n 的值为．答案：51．解：注意到0(1)C 1)nnr n r r nr x x -=+=å．其中4n x -项仅出现在求和指标4r =时的展开式444C 1)n n x-中，其4n x -项系数为44(1)(2)(3)(1)C 24n n n n n ----=．而xy 项仅出现在求和指标1r n =-时的展开式11C 1)n n nx --⋅中，其xy 项系数为12331C C 4(1)(1)2(1)(2)n n n n n n n n ----⋅-=---．因此有3(1)(2)(3)(1)2(1)(2)24n n n n n n n n ----=---．注意到4n >，化简得33(1)48n n --=-，故只能是n 为奇数且348n -=．解得51n =．7. 在平面直角坐标系中，若以(1,0)r +为圆心、r 为半径的圆上存在一点(,)a b 满足24b a ³，则r 的最小值为．答案：4．解：由条件知222(1)a r b r --+=，故22224(1)2(1)(1)a b r a r r a a £=---=---．即22(1)210a r a r --++£．上述关于a 的一元二次不等式有解，故判别式2(2(1))4(21)4(4)0r r r r --+=-³，解得4r ³．经检验，当4r =时，(,)(3,a b =满足条件．因此r 的最小值为4．8. 设等差数列{}n a 的各项均为整数，首项12019a =，且对任意正整数n ，总存在正整数m ，使得12n m a a a a +++=．这样的数列{}n a 的个数为．答案：5．解：设{}n a 的公差为d ．由条件知12k a a a +=（k 是某个正整数），则 112(1)a d a k d +=+-，即1(2)k d a -=，因此必有2k ¹，且12ad k =-．这样就有1111(1)2n n a a n d a a k -=+-=+-，而此时对任意正整数n ，12111(1)(1)(1)22n n n n n a a a a n d a n a d --+++=+=+-+ 1(1)(1)(2)2n n a n k d æö-÷ç=+--+÷ç÷çèø，确实为{}n a 中的一项．因此，仅需考虑使12|k a -成立的正整数k 的个数．注意到2019为两个素数3与673之积，易知2k -可取1,1,3,673,2019-这5个值，对应得到5个满足条件的等差数列．二、解答题：本大题共3小题，满分56分．解答应写出文字说明、证明过程或演算步骤．9.（本题满分16分）在椭圆G 中，F 为一个焦点，,A B 为两个顶点．若3,2FA FB ==，求AB 的所有可能值．解：不妨设平面直角坐标系中椭圆G 的标准方程为22221(0)x y a b a b+=>>，并记c =F 为G 的右焦点．易知F 到G 的左顶点的距离为a c +，到右顶点的距离为a c -，到上、下顶点的距离均为a ．分以下情况讨论：(1) ,A B 分别为左、右顶点．此时3,2a c a c +=-=，故25AB a ==（相应地，2()()6b a c a c =+-=，G 的方程为2241256x y +=）． …………………4分(2) A 为左顶点，B 为上顶点或下顶点．此时3,2a c a +==，故1c =，进而2223b a c =-=，所以AB ==G 的方程为22143x y +=）． …………………8分 (3) A 为上顶点或下顶点，B 为右顶点．此时3,2a a c =-=，故1c =，进而2228b a c =-=，所以AB ==G 的方程为22198x y +=）．…………………12分综上可知，AB的所有可能值为5,． …………………16分10. （本题满分20分）设,,a b c 均大于1，满足lg log 3,lg log 4.b a a c b c ì+=ïïíï+=ïî求lg lg a c ⋅的最大值．解：设lg ,lg ,lg a x b y c z ===，由,,1a b c >可知,,0x y z >．由条件及换底公式知3,4z zx y y x+=+=，即34xy z y x +==．…………………5分由此，令3,4(0)x t y t t ==>，则241212z x xy t t =-=-．其中由0z >可知(0,1)t Î． …………………10分因此，结合三元平均值不等式得2lg lg 312(1)18(22)a c xz t t t t t ==⋅-=⋅-33(22)2161818333t t t æöæö++-÷çç£⋅=⋅=÷çç÷ççèèø．当22t t =-，即23t =（相应的,,a b c 分别为8833100,10,10）时，lg lg a c 取到最大值163． …………………20分11. （本题满分20分）设复数数列{}n z 满足：11z =，且对任意正整数n ，均有2211420n n n n z z z z ++++=．证明：对任意正整数m ，均有123m z z z +++<．证明：归纳地可知*0()n z n N ¹Î．由条件得2*114210()n n n n z z n z z N ++æöæö÷çç÷++=Îçç÷çç÷èøèø，解得*11()4N n n z n z +-=Î． …………………5分因此1112n n nnz z z z ++===，故*11111()22N n n n z z n --=⋅=Î． ①进而有*11111()22N n n n n n n n z z z z n z ++-+=⋅+==Î． ②…………………10分当m 为偶数时，设*2()N m s s =Î．利用②可得122122122111123sm k k k k k k k k z z z z z z z ¥¥---===+++£+<+==ååå． …………………15分当m 为奇数时，设21()N m s s =+Î．由①、②可知21212221211112322s k k s s k k s k s z z z ¥¥+---=+=+=<==+⋅åå，故1221221212113s m k k s k k k k z z z z z z z z ¥-+-==æö÷ç+++£++<+=÷ç÷çèøåå．综上，结论获证． …………………20分。