英文版微积分考试答案
托马斯微积分ThomasCALCULUS课后习题答案附录

结束
外摆线 (圆外旋轮线) 族
x
(a
b)
cos
t
b
cos
ab b
t
y
(a
b)
sin
t
b
sin
ab b
t
center of the stationary circle (0,0), radius radius of the moving circle b,
a,
m ba
m = 1cardห้องสมุดไป่ตู้oid
m2
播点
• 轨迹:
放击 开图
M 是半径为 a 的母圆上的动点 ,
始片 或任
满足 OM = PQ 之点 P 的轨迹即为
暂意
蔓叶线
停处
• 渐近线:
• 曲线与渐近线之间的面积:
结束
folium笛卡儿叶形线
x
3at 1 t3
y
3at 2 1 t3
t 1
动点 画击 开图 始中 或任 暂意 停点
动画走向: -∞→-1 -1→+∞
Mo
x
2 a
•弧 长:
LOM
a 2
(
2 1 arsh )
其中arsh ln( 1 2 )
3
• 曲率半径 :
R
a
( 2 1)2 22
• 扇形 M 1OM 2 的面积 :
S
1 6
a
2
(
2 1
2 2
)
结束
Logarithmic
curve 对数螺线
(等角螺线) r ea
• 等角性 : 曲线与所有过极点的射线
(9) cardioid心形线
微积分英文版第九版课后练习题含答案

微积分英文版第九版课后练习题含答案微积分是数学中重要的一部分,其关注的是一条曲线或曲面的局部性质。
美国著名数学家Stewart所著的微积分英文版第九版是微积分学习的重要教材之一,本文将介绍其课后练习题,并提供答案供大家进行自我学习和测试。
课后练习题微积分英文版第九版的课后练习题共分为两部分,其中Part 1是选择题,Part 2是填空题和证明题,共计约1700道题目。
Part 1中包含了大量的选择题,这些题目主要考察读者对微积分理论的掌握和应用。
大多数题目都要求读者用所学知识推理或计算来获得正确答案。
这些题目中难易程度参差不齐,有一些比较简单,但也有一些比较困难。
Part 2的题目类型较多,包括了填空题、证明题、计算题等。
这些题目内容繁杂、难度较大,需要读者花费很多时间和精力来解答。
这些题目主要是为了检测读者对所学知识的深层次理解和应用能力,考察读者的逻辑思维和推理能力。
答案微积分英文版第九版提供了相应的课后练习答案,可以帮助读者检验自己的答案是否正确,同时也可以帮助读者更好地理解和掌握所学知识。
答案分为Part 1和Part 2两部分,且每部分分别包含了选择题和非选择题的答案。
这些答案详细、准确,提供了完整的解题思路和方法,帮助读者更好地理解题目的解法,并弥补了部分教材中的不足之处。
读者可以通过该教材的官方网站或者其他渠道获得课后练习题的答案。
结论微积分是数学中非常重要的一门学科,对于各个领域的科学研究、技术发展和社会进步都有着举足轻重的作用。
而微积分英文版第九版课后练习题则是培养和检验读者对微积分学习的深刻理解和应用能力的重要途径。
通过对这些题目的研究和答案的掌握,可以帮助读者更好地掌握微积分学科,提升自己的学术能力和科研能力。
英文版微积分考试样题3

Problems 37 - 39: Solve the problem. Assume projectile is ideal, launch angle is measured from the horizontal, and launch is over a horizontal surface, unless stated otherwise. 24) An ideal projectile is launched from level ground at a launch angle of 26° and an initial speed of 48 m/sec. How far away from the launch point does the projectile hit the ground? A) ≈ 60 m B) ≈ 230 m C) ≈ 290 m D) ≈ 185 m 25) A projectile is fired with an initial speed of 528 m/sec at an angle of 45°. What is the greatest height reached by the projectile? Round answer to the nearest tenth of a meter. A) 7111.8 m B) 76.2 m C) 69,696.0 m D) 28,447.3 m Find the unit tangent vector of the given curve. 26) r(t) = (5 + 2t7 )i + (4 + 10t7 )j + (8 + 11t7 )k A) T(t) = C) T(t) = 2 2 11 i+ j+ k 15 3 15 2 2 11 i+ j+ k 225 45 225 B) T (t) = 14 14 77 i+ j+ k 15 3 15
高三英语微积分基础单选题20题及答案

高三英语微积分基础单选题20题及答案1. In calculus, the derivative of a constant is _____.A. zeroB. oneC. itselfD. undefined答案:A。
常数的导数是零。
选项B“one”错误,常数的导数不是1。
选项C“itself”错误,常数的导数不是它本身。
选项D“undefined”错误,常数的导数是确定的,为零。
2. The process of finding the derivative is called _____.A. integrationB. differentiationC. summationD. multiplication答案:B。
求导数的过程叫做微分。
选项A“integration”是积分。
选项C“summation”是求和。
选项D“multiplication”是乘法。
3. If y = x², then the derivative of y with respect to x is _____.A. 2xB. x²C. 2x²D. x/2答案:A。
y = x²的导数是2x。
选项B“x²”错误,不是它本身。
选项C“2x²”错误,系数错误。
选项D“x/2”错误,计算错误。
4. The integral of a constant times a function is equal to the constant times the integral of the function. This is known as _____.A. the power ruleB. the product ruleC. the chain ruleD. the constant multiple rule答案:D。
常数乘以函数的积分等于常数乘以函数的积分,这被称为常数倍数法则。
高三英语微积分基础单选题40题

高三英语微积分基础单选题40题1. In calculus, the derivative of a constant is _____.A.zeroB.oneC.twoD.three答案解析:A。
在微积分中,常数的导数为零。
选项B、C、D 分别为一、二、三,都不符合常数导数的定义。
2. The integral of x with respect to x is _____.A.xB.x squaredC.x cubedD.x to the fourth power答案解析:B。
对x 积分,结果是x 的平方的一半加上常数C,但这里只考虑积分结果不考虑常数项,所以答案是x 平方。
选项A、C、D 分别为x、x 的立方、x 的四次方,都不是对x 的积分结果。
3. If y = 3x^2 + 2x + 1, then the derivative of y with respect to x is _____.A.6x + 2B.6x - 2C.3x + 2D.3x - 2答案解析:A。
对y = 3x^2 + 2x + 1 求导,3x^2 的导数是6x,2x 的导数是2,1 的导数是0,所以y 的导数是6x + 2。
选项B、C、D 分别为6x - 2、3x + 2、3x - 2,都不符合求导结果。
4. The derivative of sin(x) is _____.A.cos(x)B.-cos(x)C.sin(x)D.-sin(x)答案解析:A。
sin(x)的导数是cos(x)。
选项B、C、D 分别为-cos(x)、sin(x)、-sin(x),都不是sin(x)的导数。
5. The integral of cos(x) with respect to x is _____.A.sin(x)B.-sin(x)C.cos(x)D.-cos(x)答案解析:A。
对cos(x)积分,结果是sin(x)加上常数C,但这里只考虑积分结果不考虑常数项,所以答案是sin(x)。
山东财经大学大二经济学专业微积分英文试卷及答案2
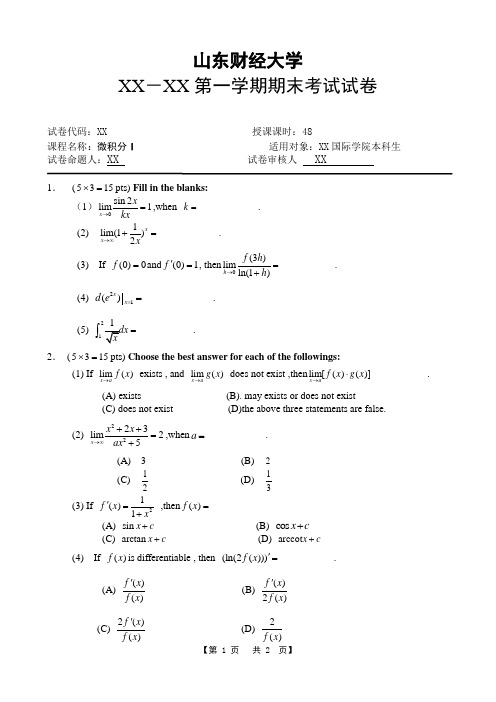
山东财经大学XX -XX 第一学期期末考试试卷试卷代码:XX 授课课时:48课程名称:微积分I 适用对象:XX 国际学院本科生 试卷命题人:XX 试卷审核人 XX1. (1535=⨯pts) Fill in the blanks:(1)0sin 2lim 1x x kx→=,when k =____________. (2) 1lim(1)2x x x→∞+=____________. (3) If (0)0f =and (0)1f '=, then 0(3)limln(1)h f h h →=+___________. (4) 21()x x d e ==______________. (5) 21=⎰___________. 2. (1535=⨯pts) Choose the best answer for each of the followings:(1) If lim ()x a f x → exists , and lim ()x a g x → does not exist ,then lim[()()]x af xg x →⋅___________. (A) exists (B). may exists or does not exist(C) does not exist (D)the above three statements are false. (2) 2223lim 25x x x ax →∞++=+,when a = ___________. (A) 3 (B) 2(C) 12(D) 13 (3) If 21()1f x x '=+ ,then ()f x =_________ (A) sin x c + (B) cos x c +(C) arctan x c + (D) arccot x c +(4) If ()f x is differentiable , then (ln(2()))f x '=___________.(A) ()()f x f x ' (B) ()2()f x f x ' (C)2()()f x f x ' (D) 2()f x(5) If 20()x F x =⎰, then )(x F '___________.(A) (B)2(C) 23.(7pts) Evaluate 4214lim x x e x x→∞-- 4.(9pts) Evaluate 210lim (cos )x x x +→. 5.(8pts)Find the derivative of the function 211()sin f x x x-=. 6.(10pts) Calculate y '' given that y is defined implicitly as a differentiable function of x by theequation x e y x y =+.7.(8pts) Find A and B given that 1()1x f x Ax B x ≤=+>⎪⎩ is differentiable everywhere. 8.(8pts)Determine the production level that will maximize the profit for a company with cost andprice functions ()1002C x x =+ and ()30p x x =-.9.(12pts) Sketch the graph of 22(1)x y x -=-. 10.(8pts) Suppose that )(x f is continuous on ]1,0[ and differentiable on )1,0(, and0)1()0(==f f . Show that there is at least a number (0,1)c ∈such that()sin ()0f c c f c '-⋅=山东财经大学XX -XX 第一学期 期末考试参考答案与评分标准 试卷代码:XX 授课课时:48课程名称:微积分Ⅰ 适用对象:XX 级国际学院2. (1535=⨯pts) Fill in the blanks:(1)2k = (2) 12e (3) 3 (4) 22e dx(5) 1)2. (1535=⨯pts) Choose the best answer for each of the followings:(1) B (2) C (3) C (4) A (5) B3.(7pts)4421444lim lim 3216lim 222x x x x xx e x e x xe →∞→∞→∞---===∞分分分 4.(9pts)2200ln(cos )1lim 01(sin )cos lim 212lim(cos )333x x x x x x x x x x e ee +→++→→⋅--===分分分. 5.(8pts)122111()2sin ()612sin 8f x x x x x x x --'=+-=分分.6.(10pts) 2214161()(1)(1)8(1)()(1)(1)10(1)x x x xx x x x xx x x x x xx e y e y y e y y e e y e y e e y e y e e y e y e e y e e ''+=+-'=-'+-+-⋅''=-'+-+-⋅=-分分分分 7.(8pts)11111lim()1lim (1)24lim 5()2lim lim 7118x x x x x Ax B A B f A B Ax B Ax A A x x A B +--++→→→→→+=+==+==+--==--==分分分分分 8.(8pts). 22()()302()()()281002()2280228()202R x xp x x x P x R x C x x x P x x x P x ==-=-=-+-'=-+==''=-<分分分分9. (12pts) 222211223334422lim0lim 0(1)(1)22lim lim 2(1)(1)11144()1(1)042122(6)066x x x x x x x x x x x x x y x x x x xy x x y x x x y x +-→∞→-∞→→--==----=-∞=-∞---'=-=-+=--'==-''=-=''==分分.10.(8pts) Setting auxiliary function cos ()()x F x e f x -=⋅, …… 2’ Thus, by the known conditions we have that )(x F is continuous on [0,1]and differentiable on (0,1), and (0)(1)0F F ==. …… 5’Hence, there exists one number at least (0,1)c ∈for which 0)(='c F by Rolle ’s theorem,cos ()sin ()0c f c c f c '⋅-⋅=. That is ()sin ()0f c c f c '-⋅= …… 8’。
英文版-微积分试卷答案

1、 (1) sin 2lim x x x→∞= 0 . (2) d(arctan )x = 1/(1+x^2) . (3) 21d sin x x =⎰ -cotx .(4).2()()x n e = 泰勒展开式(书上有。
) .(5)0x =⎰ 26/3 .2、(6) The right proposition in the following propositions is ____A____.A. If lim ()x a f x →exists and lim ()x a g x →does not exist then lim(()())x af xg x →+does not exist. B. If lim ()x a f x →,lim ()x a g x →do both not exist then lim(()())x af xg x →+does not exist. C. If lim ()x a f x →exists and lim ()x a g x →does not exist then lim ()()x af xg x →does not exist. D. If lim ()x a f x →exists and lim ()x a g x →does not exist then ()lim ()x a f x g x →does not exist. (7) The right proposition in the following propositions is __A______.A. If lim ()()x af x f a →=then ()f a 'exists. B. If lim ()()x af x f a →≠ then ()f a 'does not exist. C. If ()f a 'does not exist then lim ()()x af x f a →≠. D. If ()f a 'does not exist then the cure ()y f x =does not have tangent at (,())a f a .(8) The right statement in the following statements is __D ______. A. sin lim 1x x x→∞= B. 1lim(1)x x x e →∞+= C. 11d 1x x x C ααα+=++⎰ D. 5511d d 11bb a a x y x y =++⎰⎰ (9) For continuous function ()f x , the erroneous expression in the following expressions is __C ____. A.d (()d )()d b a f x x f b b =⎰ B. d (()d )()d b af x x f a a =-⎰ C. d (()d )0d b a f x x x =⎰ D. d (()d )()()d b af x x f b f a x =-⎰ (10) The right proposition in the following propositions is ____D____. A. If ()f x is discontinuous on [,]a b then ()f x is unbounded on [,]a b .B. If ()f x is unbounded on [,]a b then ()f x is discontinuous on [,]a b .C. If ()f x is bounded on [,]a b then ()f x is continuous on [,]a b .D. If ()f x has absolute extreme values on [,]a b then ()f x is continuous on [,]a b .3、Evaluate 2011lim()x x e x x→-- 1/24.Find 0d |x y =and (0)y ''if 20x x x y y t e +=+⎰. 隐函数求导。
TB_chapter2

1. The point ()2,4P lies on the curve x y =. If Q is the point (x , use your calculator to find the slope of the secant line PQ (correct to six decimal places) for the value .99.3=x2. The displacement (in meters) of an object moving in a straight line is given by 212/4s t t =++, where t ismeasured in seconds. Find the average velocity over the time period [1,3].3.Find the limit.()379lim 25++→x x x4.Find the limit.()51lim 20+-→x x x x5. If ,22)(12++≤≤x x x f for all x find the limit.)(lim 1x f x -→6. Find the limit. 22lim |2|x x x →--7. Evaluate the limit.()xx x 11022lim --→-+8. Use the definition of the derivative to find (2)f '-, where 3()2f x x x =-.9. Find an equation of the tangent line to curve 32y x x =-at the point (2,4).10. Use a graph to find a number N such that 3.031235622<---+x x x whenever N x >.11. If ()g x ()g x '.12. A machinist is required to manufacture a circular metal disk with area 21000cm .a) What radius produces such a disk? b) If the machinist is allowed an error tolerance of 25cm ±in the area of the disk, how close to the idealradius in part (a) must the machinist control the radius?13. Use a graph to find a number δ such that6.0314<-+x whenever .2δ<-x14. For the limit, illustrate the definition by finding values of δ that correspond to .25.0=ε31lim(43)2x x x →+-=15. Determine where f is discontinuous.()20()30333if x f x x if x x if x ⎧<⎪⎪=-≤<⎨⎪->⎪⎩16. For x = 5, determine whether f is continuous from the right, from the left, or neither.17. If a cylindrical tank holds 100,000 gallons of water, which can be drained from the bottom of the tank in an hour,then Torricelli's Law gives the volume of water remaining in the tank after t minutes as2651000,100)(⎪⎭⎫ ⎝⎛-=t t V , 600≤≤tFind the rate at which the water is flowing out of the tank (the instantaneous rate of change of V with respect to t ) as a function of t .18.Find the derivative of the function.25314)(x x x f +-=19. If 2313)(tt f += find )(t f '.20. At what point is the function ()|6|f x x =- not differentiable.ANSWER KEYStewart - Calculus ET 6e Chapter 2 Form A1. 0.2501562. 3/m s3. 2634. -∞5. 16. Limit does not exist7. -1/48. 109. 1016y x =-10. 9≥N11.(),3/5-∞12. cm , 0.0445cm13. 81.0≤δ14. 030.0≤δ15. 03at and16. neither17. ⎪⎭⎫ ⎝⎛--=65165200000t y 18. 310-x19. )3(26t t +- 20. 61.The point P (4, 2) lies on the curve .x y = If Q is the point ()x x ,, use your calculator to find the slope of the secant line PQ (correct to six decimal places) for the value of .01.4=x2.The displacement (in meters) of an object moving in a straight line is given by 212/4s t t =++, where t is measured in seconds. Find the average velocity over the time period [1,1.5].3.Find the limit, if it exists.44lim |4|x x x →-- 4. Find )(a f '.252)(x x x f -+=5. Find an equation of the tangent line to curve 32y x x =-at the point (2,4).6. Evaluate the function 222)(--=x x x f at the given numbers (correct to six decimal places). Use the results to guess the value of the limit ).(lim 2x f x →7. The graph of f is given. State the numbers at which f is not differentiable.⎪⎭⎫ ⎝⎛→x x x 3cos lim 909. If 66)(12++≤≤x x x f for all x find the limit.)(lim 1x f x -→10.Evaluate the limit.()867lim 25++→x x x11. Evaluate the limit.()x x x 11022lim --→-+12.If an arrow is shot upward on the moon, with a velocity of 70 m/s its height (in meters) after t seconds is given by .99.070)(2t t t H -= With what velocity will the arrow hit the moon?13. The cost (in dollars) of producing x units of a certain commodity is .08.013336,4)(2x x x C ++= Find the average rate of change with respect to x when the production level is changed from 101=x to .103=x14.Let ()20()30333if x f x xif x x if x ⎧<⎪⎪=-≤<⎨⎪->⎪⎩Evaluate each limit, if it exists.0lim ()x f x +→ b.) 0lim ()x f x -→15.If f and g are continuous functions with 3)3(=f and []3)()(3lim 3=-→x g x f x , find ).3(g16.Evaluate the limit. 9lim 9+-→x x17.Find a number δsuch that if |2|x δ-<, then |48|x ε-<, where 0.1ε=.then Torricelli's Law gives the volume of water remaining in the tank after t minutes as2651000,100)(⎪⎭⎫ ⎝⎛-=t t V , 600≤≤tFind the rate at which the water is flowing out of the tank (the instantaneous rate of change of V with respect to t ) as a function of t .19. If ()g x ()g x '.20. For the function f whose graph is shown, state the following.)(lim 4x f x -→ANSWER KEYStewart - Calculus ET 6e Chapter 2 Form B1. 0.2498442.2.625 m/s3. Limit does not exist4. a 101-5.1016y x =- 6.(1.6, 0.7465), (1.8, 0.7257), (1.9, 0.7161), (1.99, 0.7079), (1.999, 0.707195), (2.4, 0.674899), (2.2, 0.690261), (2.1, 0.698482), (2.01, 0.706225), (2.001, 0.707018), Limit = 0.707107 7. 1,0,3-8. 09. 110. 21311. -1/412. -7013. 29.3214. a.) 3 b.) 015. 616. 017. 0.025δ=18. ⎪⎭⎫ ⎝⎛--=65165200000t y 19. (),3/5-∞20. -∞1. A cardiac monitor is used to measure the heart rate of a patient after surgery. It compiles the number of heartbeats after t minutes. When the data in the table are graphed, the slope of the tangent line represents the heart rate inbeats per minute. The monitor estimates this value by calculating the slope of a secant line. Use the data to estimate the patient's heart rate after 42 minutes using the secant line between the points with t = 38 and t = 42.Select the correct answer.a. -89b. 180c. 90d. 100e. 89f. 952. If an arrow is shot upward on the moon with a velocity of 55 m/s, its height in meters after t seconds is given by .04.0552t t h -= Find the average velocity over the interval [1, 1.04].Select the correct answer.a. 54.9194b. 55.0284c. 54.8174d. 54.9184e. 54.90843. The displacement (in feet) of a certain particle moving in a straight line is given by 3/8s t = where t is measuredin seconds. Find the average velocity over the interval [1, 1.8].Select the correct answer.a. 0.865b. 0.654c. 0.765d. 0.756e. 0.745f. 0.7554. For the function f whose graph is shown, find the equations of the vertical asymptotes.Select all that apply.a. x = -7b. x = 9c. x = 5d. x = -3e. x = 10f. x = -25. Find the limit, if it exists55lim |5|x x x →--Select the correct answer.a. 5b. 1-c. 1-d. 0e. limit does not exist6. Find the limit.lim x →-∞Select the correct answer.a. -1/2b. 3c. 3-d. 0e. limit does not exist7. Evaluate the limit.()()62lim 231-+→x x xSelect the correct answer.a. 27b. -45c. -135d. 29e. -1258.If 88)(12++≤≤x x x f for all x , find )(lim 1x f x -→. Select the correct answer.a. 1b. 8c. -1/8d. -1/16e. The limit does not exist9. Evaluate the limit.⎪⎭⎫ ⎝⎛→x x x 5cos lim 90Select the correct answer.a. -5b. 1c. 0d. 5e. The limit does not exist10. Use a graph to find a number δ such that 2.021sin <-x whenever δπ<-6x .Round down the answer to the nearest thousandth.Select the correct answer.a. 218.0≤δb. 368.0≤δc. 401.0≤δd. 251.0≤δe. 425.0≤δ11. A machinist is required to manufacture a circular metal disk with area 1000 cm 2. If the machinist is allowed an error tolerance of ±10 cm 2 in the area of the disk, how close to the ideal radius must the machinist control the radius?Round down the answer to the nearest hundred thousandth.Select the correct answer.a. cm 08898.0≤δb. cm 08908.0≤δc. cm 08999.0≤δd. cm 08913.0≤δe. cm 09913.0≤δ12. Consider the function x e x f /121)(+=. Find the value of -→0)(lim x x f . Select the correct answer.a. 1.5b. -0.1c. 0.1d. 0.9e. 0.513.Choose an equation from the following that expresses the fact that a function f is continuous at the number 6.Select the correct answer.a. 6)(lim =∞→x x fb. )6()(lim 6f x f x =→c. )6()(lim f x f x =∞→d. 0)(lim 6=→x x fe. ∞=→6)(lim x x f14. Determine where f is discontinuous.()20()30333if x f x x if x x if x ⎧<⎪⎪=-≤<⎨⎪->⎪⎩Select the correct answer.a. 03andb. 0onlyc. 3onlyd. 03and -e. 3only -15. Use the definition of the derivative for find (2)f '-, where 3()2f x x x =-.Select the correct answer.a. 4b. 10c. -4d. -10e. none of these16.If ()g x ()g x '.Select the correct answer.a. ()(),00,-∞⋃∞b. [3/5,3/5]-c. [,3/5)-∞d. (),3/5-∞e. ()0,∞17. Find an equation of the tangent line to the curve 353+-=x x y at the point (2, 1).Select the correct answer.a. 138+=x yb. 139--=x yc. 137-=x yd. 137+-=x ye. 157-=x yStewart - Calculus ET 6e Chapter 2 Form C18. The cost (in dollars) of producing x units of a certain commodity is 201.019571,4)(x x x C ++=. Find theinstantaneous rate of change with respect to x when x = 103. (This is called the marginal cost .)Select the correct answer.a. 26.06b. 20.06c. 21.06d. 18.06e. 31.0619. If the tangent line to )(x f y = at (8, 4) passes through the point (5, -32), find )8(f '.Select the correct answer.a. 24)8(='fb. 20)8(='fc. 12)8(-='fd. 12)8(='fe. 32)8(='f20. At what point is the function ()|6|f x x =- not differentiable.Select the correct answer.a. 6b. 6-c. 1d. 1-e. 0ANSWER KEYStewart - Calculus ET 6e Chapter 2 Form C1. c2. d3. f4.a, b, c, f5. e6. a7. c8. a9. c10. a11. a12. e13. b14. a15. b16. d17. c18. c19. d20. a1. The position of a car is given by the values in the following table.Find the average velocity for the time period beginning when t = 2 and lasting 2 seconds.Select the correct answer.a. 35.5b. 47.5c. 39d. 37.5e. 33.52. The displacement (in meters) of an object moving in a straight line is given by 212/4s t t =++, where t ismeasured in seconds. Find the average velocity over the time period [1,3].Select the correct answer.a. 3/m sb. 3.5/m sc. 1/m sd. 1.5/m se. none of these3. Find the limit.()71lim 20++→x x x xSelect the correct answer.a. 0b. 71c. 71- d. -∞ e. ∞4. Find the limit.lim x →-∞Select the correct answer.a. -1b. 0c. 1/2d. -∞e. -1/25.The slope of the tangent line to the graph of the exponential function xy 8= at the point (0, 1) is x x x 18lim 0-→. Estimate the slope to three decimal places.Select the correct answer.a. 1.293b. 2c. 2.026d. 1.568e. 2.079f. 2.5566. Find an equation of the tangent line to curve 32y x x =-at the point (2,4).Select the correct answer.a. 1610y x =-b. 108y x =-c. 16y x =-d. 1016y x =+e. none of these7.Find the limit.()10lim tan 1/x x +-→Select the correct answer.a. 0b. ∞c. /2πd. /3πe. π .8. Let |1|1)(2--=x x x F . Find the following limits.),(lim 1x F x +→ )(lim 1x F x -→Select the correct answer.a. both 2b. 2 and 1c. 2 and – 2d. 2 and – 1e. both 19. Use continuity to evaluate the limit.()x x x sin 4sin lim 13+→πSelect the correct answer.a. π13b. - 1c. 0d. ∞e. 110.Let ()20()30333if x f x xif x x if x ⎧<⎪⎪=-≤<⎨⎪->⎪⎩Evaluate the limit, if it exists.0lim ()x f x -→Select the correct answer.e. 3-11. For what value of the constant c is the function f continuous on ()?,∞∞-⎩⎨⎧>-≤+=2527)(2x for cx x for cx x fSelect the correct answer.a. 1=cb. 2=cc. 6=cd. 2-=ce. 7=c12. Find a function g that agrees with f for 25≠x and is continuous on ℜ.xx x f --=255)(Select the correct answer.a. x x g -=51)(b. x x g +=251)(c. x x g +=51)(d. xx g -=51)( e. x x g -=55)(13.Which of the given functions is discontinuous?Select the correct answer.a. 5,5,3121)(<≥⎪⎩⎪⎨⎧-=x x x x fb. 5,5,351)(=≠⎪⎩⎪⎨⎧-=x x x x fc. Both functions are continuous14.Find the limit. 13lim 232-++∞→t t t tSelect the correct answer.a. ∞b. 0c. 3-d. 3e. 215.If ()g x ()g x '.Select the correct answer.a. ()(),00,-∞⋃∞b. [3/5,3/5]-c. [,3/5)-∞d. (),3/5-∞e. ()0,∞16. The cost (in dollars) of producing x units of a certain commodity is .03.013280,4)(2x x x C ++= Find theaverage rate of change with respect to x when the production level is changed from x = 102 to x = 118.Select the correct answer.a. 29.6b. 19.6c. 18.6d. 26.6e. 24.617. Evaluate the limit.|2|lim 2+-→x xSelect the correct answer.a. 2b. 4c. - 2d. 0e. The limit does not exist18. If a ball is thrown into the air with a velocity of 58 ft/s, its height (in feet) after t seconds is given by .11582t t H -=Find the velocity when t = 4.Select the correct answer.a. 27ft/sb. 30ft/sc. 31ft/sd. 25ft/se. 37ft/s19. Is there a number a such that 626lim 223-++++-→x x a ax x x exists? If so, find the value of a and the value of the limit. Select the correct answer.a. a =14, limit equals 1.4b. a =17, limit equals 1.6c. a =28, limit equals 1.4d. a =28, limit equals 1.6e. There is no such number20.If ()g x ()g x '.Select the correct answer.a. ()1/25()352g x x -'=-- b. ()1/21()352g x x '=-- c. ()2()35g x x '=-- d. ()25()352g x x -'=-- e. none of theseANSWER KEYStewart - Calculus ET 6e Chapter 2 Form D1. d2. a3. e4. e5. e6. e7. c8. c9. c10. a11. c12. c13. b14. b15. d16. b17. d18. b19. d20. a1.A tank holds 1000 gallons of water, which drains from the bottom of the tank in half an hour. The values in the table show the volume V of water remaining in the tank (in gallons) after t minutes. If P is the point (15, 263) on thegraph of V, fill the table with the slopes of the secant lines PQ where Q is the point on the graph with the corresponding t .Enter your answer to two decimal places.2. The displacement (in meters) of an object moving in a straight line is given by 212/4s t t =++, where t ismeasured in seconds. Find the average velocity over the time period [1,1.5].3. If an arrow is shot upward on the moon with a velocity of 57 m/s, its height in meters after t seconds is given by 282.057t t h -=. Find the instantaneous velocity after one second.Select the correct answer.a. 55.46b. 55.35c. 55.25d. 55.36e. 55.374. Given that, 3)(lim 7-=→x f x and 9)(lim 7=→xg x . Evaluate the limit.)()()(2lim 7x f x g x f x -→5. Find an equation of the tangent line to curve 32y x x =-at the point (2,4).Select the correct answer.a. 1610y x =-b. 108y x =-c.16y x =- d. 1016y x =+ e. none of these6. Let ()20()30333if x f x x if x x if x ⎧<⎪⎪=-≤<⎨⎪->⎪⎩Evaluate the limit, if it exists.0lim ()x f x -→Select the correct answer.e. 3-7. For the function f whose graph is shown, find the following.)(lim 7x f x →8.For x = 5, determine whether f is continuous from the right, from the left, or neither.9. Evaluate the limit.()xx x 11077lim --→-+10. Let |1|1)(2--=x x x FFind the following limits.)(lim ),(lim 11x F x F x x -+→→11. Use a graph to find a number δsuch that 3|0.6< whenever |2|x δ-<.Round down the answer to the nearest hundredth.12. Is there a number a such that 6810lim 223-++++-→x x a ax x x exists? If so, find the value of a and the value of the limit.Select the correct answer.a. a =49, limit equals 1.6b. a =13, limit equals 2.2c. a =49, limit equals 2.2d. a =19, limit equals 1.6e. a =49, limit equals 2.713. How close to 2 do we have to take x so that 5x + 3 is within a distance of 0.025 from 13?14. Find a function g that agrees with f for 25≠x and is continuous on .ℜxx x f --=255)( 15. Use the given graph of x x f =)( to find a number δ such that 4.0|2|<-x whenever .|4|δ<-x16.If ()g x ()g x '.17.If ()g x ()g x '.Select the correct answer.a. ()(),00,-∞⋃∞b. [3/5,3/5]-c. [,3/5]-∞d. (),3/5-∞e. ()0,∞18. At what point is the function |6|)(x x f -= not differentiable.19. How close to - 9 do we have to take x so that ()?10000914>+x20.Find the derivative of the function.25314)(x x x f +-=ANSWER KEYStewart - Calculus ET 6e Chapter 2 Form E1. 5, -42.3, 10, -43.6,20, -18.4, 25, -24.1, 30, -17.532. 2.625 m/s3.d 4.-1/2 5. e6. a7.-∞ 8.neither 9. -1/4910. 2, -211. 81.0≤δ12. c13. 005.0|2|<-x14. ()x g +=5115. 44.1≤δ 16. ()1/25()352g x x -'=-- 17. d18. 619. 1.0|9|<+x20. 310-x1. The displacement (in meters) of an object moving in a straight line is given by 212/4s t t =++, where t ismeasured in seconds. Find the average velocity over the time period [1,1.5].2. If a ball is thrown into the air with a velocity of 45 ft/s, its height in feet after t seconds is given by 21545t t y -=. Find the instantaneous velocity when 4=t .3. If 5.4)(lim 3=-→x f x , then if )(lim 3x f x → exists, to what value does it converge?Select the correct answer.a. 6.5b. 4.5c. 1d. 2e. 64. For the function f whose graph is shown, find the limit.)(lim 9x f x +-→5. The function has been evaluated at the given numbers (correct to six decimal places). Use the results to guess the value of the limit.112)(--=x x x f________)(lim 1=→x f xSelect the correct answer.a. 1.255039b. 1.911314c. 1.969944d. 1.473889e. 16.Evaluate the limit.()()104lim 251-+→x x x7. Find the limit.lim x →-∞8.Find the limit.()10lim tan 1/x x +-→9.Evaluate the limit and justify each step by indicating the appropriate properties of limits.393198lim 22-++-∞→x x x x x10. Find an equation of the tangent line to the curve 34x y =at the point ()256,4--.11. Find a number δsuch that if |2|x δ-<, then |48|x ε-<, where 0.1ε=.12. Use a graph to find a number δsuch that 1.021sin <-x whenever δπ<-6x .Round down the answer to the nearest thousandth.13. Use the definition of the limit to find values of δ that correspond to 75.0=ε.Round down the answer to the nearest thousandth.()234lim 31=-+→x x x14. Determine where f is discontinuous.()20()30333if x f x x if x x if x ⎧<⎪⎪=-≤<⎨⎪->⎪⎩15. If f and g are continuous functions with 2)2(=f and [],2)()(2lim 2=-→x g x f x find )2(g .16. Find the limit.)(lim 22bx x ax x x +-+∞→17.State the domain.()sin F x =18.Find the derivative of the function using the definition of derivative.22919)(x x x f +-=19. Find a function g that agrees with f for 4≠x and is continuous on ℜ.xx x f --=42)(20. If an arrow is shot upward on the moon, with a velocity of 70 m/s its height (in meters) after t seconds is given by.99.070)(2t t t H -= With what velocity will the arrow hit the moon?ANSWER KEYStewart - Calculus ET 6e Chapter 2 Form F1. 2.625 m/s2. -753. b4. -∞5. e6. 28125-7. -1/28.2π 9. 38 10. 512192+=x y11. 0.025δ=12. 112.0≤δ13. 085.0≤δ14.03at and 15.2 16. 2b a - 17. ),6[∞18.94-x 19.x g +=2120.-701. If 5.4)(lim 2=-→x f x , then if )(lim 2x f x →exists, to what value does it converge?Select the correct answer.a. 2b. 1c. 5d. 4.5e. 1.52. Consider the following function.()111111)(2≥<≤--<⎪⎩⎪⎨⎧--=x x x x x x x fDetermine the values of a for which )(lim x f ax →exists.3. Evaluate the limit and justify each step by indicating the appropriate properties of limits.443398lim 22-++-∞→x x x x x4. Find )(a f '.233)(x x x f -+=5. Guess the value of the limit.3055tan 3lim xx x x -→Select the correct answer.a. 121b. 135c. 134d. 130e. 1256. Given that 8)(lim 7-=→x f x and 10)(lim 7=→x g x .Evaluate the limit.())()(lim 7x g x f x +→7.Evaluate the limit.()()101lim 231-+→x x x8. Evaluate the limit.⎪⎪⎭⎫⎝⎛--→45lim 233x x x9. Find the derivative of the function using the definition of the derivative.2610)(x x x f +-=10.Let |9|81)(2--=x x x FFind the following limits.)(lim ),(lim 99x F x F x x -+→→Select the correct answer.a. 18 and 9b. 18 and - 18c. both 18d. 18 and – 9e. 81 and 911.Use the given graph of x x f =)(to find a number δsuch that 4.0|2|<-x whenever .|4|δ<-x12. Use a graph to find a number δsuch that 5.0|314|<-+x whenever .|2|δ<-x13. For the limit, illustrate the definition by finding values of δthat correspond to .5.0=ε()234lim 31=-+→x x x14. Find the slope of the tangent line to the curve 35x y = at the point (-4, -320).15. At what point is the function |8|)(x x f -= not differentiable.16.Which of the given functions is discontinuous?a. 5,5,3121)(<≥⎪⎩⎪⎨⎧-=x x x x f b. 5,5,351)(=≠⎪⎩⎪⎨⎧-=x x x x fc. Both functions are continuous17.Select the right number for the following limit and prove the statement using the ,δε definition of the limit. 3183lim 23--+→x x x xSelect the correct answer.a. 6b. 8c. 5d. 9e. 1818.Prove the statement using the ,δε definition of the limit.0|2|lim 2=-→x x19.Prove the statement using the ,δε definition of the limit.()241lim 25=--→x x20.Use continuity to evaluate the limit.()x x x sin 3sin lim 17+-→πSelect the correct answer. a. π17- b. ∞ c. -1 d. 0 e. 1ANSWER KEYStewart - Calculus ET 6e Chapter 2 Form G1. d2. ()()()∞--∞-,11,11,3.38 4.a 61- 5.e 6. 27. -728.22/5 9. 112-x10. b11. 44.1≤δ12. 6875.0≤δ13. 056.0≤δ14. 24015. 816. b17. d18. Given 0>ε, we need 0>δsuch that if | x - 2 | δ< then | | x - 2 | - 0 | ε<. But | | x - 2 | | = | x - 2 |. So this is true ifwe pick .εδ=19. Given 0>ε, we need 0>δsuch that if | x - ( - 5 ) | δ< then | ( x 2 - 1 ) - 24 | ε< or upon simplifying we need | x2 – 25| ε<whenever | x + 5 | δ<. Notice that if | x + 5 | < 1 , then- 1 < x + 5 < 1 - 11 < x - 5 < - 9 | x - 5 | < 11. So take =δmin {ε / 11, 1}. Then | x - 5 | < 11 and | x + 5 | ε</ 11, so | ( x 2 - 1 ) - 24 | = | ( x + 5 ) ( x - 5 ) | = | x + 5 | | x - 5 | < (ε / 11 ) ( 11 ) =ε. Therefore, by the definition of a limit, ().241lim 25=--→x x 20.dStewart - Calculus ET 6e Chapter 2 Form H1. The point P (4, 2) lies on the curve x y =. If Qis the point (,x , use your calculator to find the slope of thesecant line PQ (correct to six decimal places) for the value of 99.3=x .Select the correct answer.a. m PQ = 0.250157b. m PQ = 0.250156c. m PQ = - 0.250154d. m PQ = - 0.250156e. m PQ = 0.2501542. The displacement (in meters) of an object moving in a straight line is given by 212/4s t t =++, where t ismeasured in seconds. Find the average velocity over the time period [1,1.5].3. The displacement (in feet) of a certain particle moving in a straight line is given by 83t s =where t is measured in seconds. Find the instantaneous velocity when t = 3.4. If ,5.7)(lim 2=+→x f x then if )(lim 2x f x →exists, to what value does it converge?Select the correct answer. a. 5 b. 8.5 c. 8 d. 11.5 e. 7.55.If f and g are continuous functions with 3)2(=f and [],5)()(3lim 2=-→x g x f x find ).2(g6. The slope of the tangent line to the graph of the exponential function xy 4=at the point (0, 1) is .14lim 0x x x -→ Estimate the slope to three decimal places. Select the correct answer.a. 1.045b. 1.136c. 0.786d. 1.126e. 1.3867. Find an equation of the tangent line to curve 32y x x =-at the point (2,4).Select the correct answer.a. 1610y x =-b. 108y x =-c. 16y x =-d. 1016y x =+e. none of these8. Find the limit.lim x →-∞9. How close to 2 do we have to take x so that 5x + 3 is within a distance of 0.075 from 13?10. Evaluate the limit and justify each step by indicating the appropriate properties of limits.693958lim 2-++-∞→x x x x x11. Find a number δsuch that if |2|x δ-<, then |48|x ε-<, where 0.01ε=.12. Use the given graph of 2)(x x f =to find a number δsuch that 2112<-x whenever δ<-1x .Round down the answer to the nearest hundredth.13.If ()g x ()g x '.14.If ()g x ()g x '.15.Let ()20()30333if x f x x if x x if x ⎧<⎪⎪=-≤<⎨⎪->⎪⎩Evaluate each limit, if it exists.a.) 0lim ()x f x +→b.) 0lim ()x f x -→16.Which of the given functions is discontinuous?Select the correct answer.a. 5,5,3121)(<≥⎪⎩⎪⎨⎧-=x x x x f b. 5,5,351)(=≠⎪⎩⎪⎨⎧-=x x x x fc. Both functions are continuous17.If a ball is thrown into the air with a velocity of 62 ft/s, its height (in feet) after t seconds is given by21662t t H -=.Find the velocity when t = 5.18.Use continuity to evaluate the limit.()x x x sin 6sin lim 8+→πSelect the correct answer.a. ∞b. - 1c. 1d. 0e. π819. Find a function g that agrees with f for 16≠x and is continuous on ℜ.xx x f --=164)( 20. Consider the function .11)(/1x e x f +=Find the value of )(lim 0x f x +→.Select the correct answer.a. -0.8b. -0.5c. 0.3d. 0e. 0.8ANSWER KEYStewart - Calculus ET 6e Chapter 2 Form H1. b2. 2.625 m/s3. 3.3754. e5. 46. e7. e8. -1/29.015.0|2|<-x 10. 38 11. 0.0025δ=12. 22.0≤δ13. ()1/25()352g x x -'=-- 14. (),3/5-∞15. a.) 3 b.) 016. b17. -9818. d19. x g +=4120. d。
英文版微积分考试样题1
9)
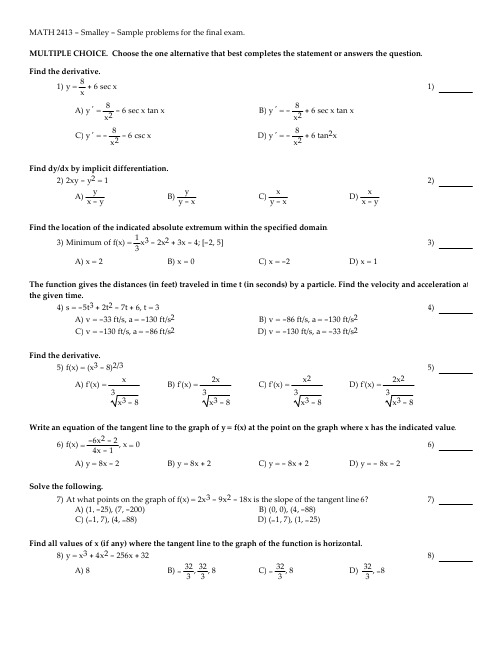
Give an appropriate answer. 10) Find the instantaneous rate of change for the function x2 + 7x at x = 2. A) 9 B) 11 C) 4 Determine whether the limit exists. If it exists, find its value. 11) lim f(x) x → nction gives the distances (in feet) traveled in time t (in seconds) by a particle. Find the velocity and acceleration at the given time. 4) s = - 5t3 + 2t2 - 7t + 6, t = 3 4) A) v = - 33 ft/s, a = - 130 ft/s2 C) v = - 130 ft/s, a = - 86 ft/s2 Find the derivative. 5) f(x) = (x3 - 8)2/3 A) f'(x) = x 3 x3 - 8 B) f'(x) = 2x 3 x3 - 8 C) f'(x) = x2 3 x3 - 8 D) f'(x) = 2x2 3 x3 - 8 B) v = - 86 ft/s, a = - 130 ft/s2 D) v = - 130 ft/s, a = -33 ft/s2
8)
Use the properties of limits to help decide whether the limit exists. If the limit exists, find its value. - 3x2 + 3x - 7 9) lim 4x2 + 3 x→∞ A) ∞ B) 3 7 C) 0 D) 3 4
英文版微积分

Infinite Sequences and Series
一、Determine whether the series is convergent or divergent
2 2 n !
(1) n 1 n n
(2)
(
n )n
n1 2n 1
2n2 3n1
(3)
(4)
1
n1 3n7 n2 2
Determine whether the series
an
n0
is convergent or divergent. And find the sum of this series.
Example
(19) 求幂级数
1 n1 x2n1
n1 n 2n 1
的收敛域及和函数
(9) 若级数
求幂级数
1 (1)n x2n (x1)
n1
2n
的和函数f(x)及其极值.
True or false
lim a 0 a 1. If
,then
n n
is convergent.
n
2.
a If
is divergent ,then
divergent. n
is
an
3.
a b If
for all ,and
z
z .
x y
x3 y3 2.If f(x,y)x2 y2
calculate
and 0
x2 y2 0 x2 y2 0
fx (0,0)
f y (0,0).
Vectors and Geometry of Space
If is the angle between the nonzero
微积分试卷答案

IEN INSTITUTE of MINJIANG UNIVERSITYAnswer Key and Marking CriterionClosed-book Exam Paper ASubject : Calculus Ⅰ 2013—2014 Academic Year Semester 1 Cohort & Major: AC1- 4 Teacher ’s Name: Chen LanqingPart I MCQ 12%1. A2. B3. C4. CPart Ⅱ Fill in blanks. 24%1. 21;2. 82 feet ;3. 45x +340;4. 6;5. 24y x =-+;6. 58;7. 1y =-;8. 5, 1 Part Ⅲ Find the following limits. 16%1. Solution121x x →-lim(21)13x -= 2. Solution545545652265lim lim221211x x x x x x x x x x→+∞→+∞+-+-==-+-+ 3.Solution221111lim lim 021x x x x x→+∞→+∞++==-- 4. Solution02)l i l l i m 42)42)x x x x x x →→→→+===-+=--Part ⅣDifferentiate the following functions and simplify your answers. 16% 1. Solution332324312()(1231342xf x xxxx xx x'-⎛⎫'=++⎪⎝⎭'⎛⎫=++-⎪⎝⎭=+-+2. Solution2222()[(3)1)]181)(3)181818f x x x xx x''=+=++=+-=+3. Solution12233222433110(32)(15)(32)(2)15509023()(32)3(32)x x x xx x xf xx x--+--+'⎛⎫----'===⎝++4. Solution322222411112()33111111116(1)61(1)(1)x x x xf xx x x x xx xx x x'⎛⎫''----⎛⎫⎛⎫⎛⎫⎛⎫⎛⎫' ⎪===-⎪ ⎪ ⎪ ⎪ ⎪⎪+++++⎝⎭⎝⎭⎝⎭⎝⎭⎝⎭⎝⎭--⎛⎫==⎪+⎝⎭++Part Ⅴ PROBLEM SOLVING 32% 1. Solution 12%The function f (x ) is defined for all x ≠-2 and has (0, 0) as its only intercept.2x =- is a vertical asymptote. There are no horizontal asymptotes. …….(2 pts)222(2)(2)(4)()(2)(2)x x x x x f x x x +-+'==++So ()0f x '= when 4,0x =-. We have the arrow diagram as follows :(-4, -8) and (0,0) are relative extrema. …………………….(8 pts)2243(2)(24)2(4)(2)8()(2)(2)x x x x x f x x x ++-++''==++So ()f x ''is not defined only for 2x =-. The concavity diagram can be shownbelow.Join those key features by a smooth curve.…………………………..(12 pts)2. Solution 10% a. We find that2()(0.04573)0.085c x x x x ''=++=+The estimated cost of producing the 410th unit is marginal cost (409)(0.08)(409)537.72thousand dollars.c '=+= b. The actual cost of producing the 410th unit is()22(410)(409)0.04(410)5(410)730.04(409)5(409)73c c -=++-++0.04(410409)537.76thousand dollars.=++=3. Solution 10%Let x be the number of mulch (in tons )in each shipment. The costs include:purchase cost (4,000)(20)80,000==4,000ordering cost (30)x ⎛⎫= ⎪⎝⎭storage cost (1.5)2x ⎛⎫= ⎪⎝⎭So, the total cost is120,000()80,0000.75C x x x=++ which is the function to be minimized on 14,000x ≤≤. 2120,000()0.75C x x'=-+()0C x '= when x = 4003240,000(),C x x''=so (400)0C ''> and there is a relative minimum when x = 400.C (400) = 80,600, C (1) = 200,000.75, C (4,000) = 83,030.So, the cost is minimized when 400 tons of mulch is ordered in each shipment.。
微积分 高等数学 英文练习题以及答案(1)

lim
n
sin n 0. n
(n 1, 2, ) 。
n
n
(4) xn sin
n ; 2
1 证:因为 sin x 1,所以 sin n n1 有界,而 lim 0 ,根 n n
据题目已知的结论有:
则 xn 1,
(n 1, 2, ) ,lim xn 1 , 但是 lim xn 不存在。
3
Exercise 1-2 Limits of Sequences 1. Observe the behavior of the general term of each of the following sequences as n increases infinitely, determine the sequences that have limits and write out the limit if it exists: (1) xn
(7) xn cos
1 ; n
极限存在,为 1。
n 4 n 4 n 4 4 1 2 0.1 2 n n n( n 4 n) 2n
2 2
只要 n 20或n 5 即可。
2
xn a n .
(8) xn ln
1 . n
所以取 N 5 , 则当 n N , xn a 即 成立。
Xi’an Jiaotong-Liverpool University
Subgroup
Name
ID No.
1
Preliminaries: Functions 1. Find the natural domains of the following functions: (1)
微积分 高等数学 英文练习题以及答案(2)
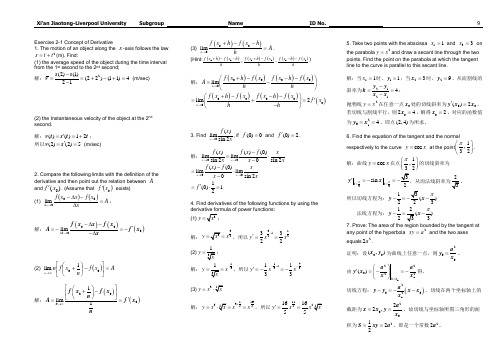
Exercise 2-1 Concept of Derivative1. The motion of an object along the s -axis follows the law2s t t =+(m). Find:(1) the average speed of the object during the time interval from the 1st second to the 2nd second; 解:2(2)(1)(22)(11)421s s v -==+-+=- (m/sec)(2) the Instantaneous velocity of the object at the 2nd second.解:()()12v t s t t '==+; 所以(2)(2)5v s '== (m/sec)2. Compare the following limits with the definition of the derivative and then point out the relation between A and 0()f x '. (Assume that ()0x f ' exists) (1) ()()A xx f x x f x =∆-∆-→∆000lim ;解:()()()0000lim x fx x fx A f x x∆→-∆-'=-=--∆(2) ()A x f n x f n n =⎥⎦⎤⎢⎣⎡-⎪⎭⎫ ⎝⎛+∞→001lim解:()()0001lim1n f x f x n A f x n→∞⎡⎤⎛⎫+- ⎪⎢⎥⎝⎭⎣⎦'==(3) ()()00limh fx h fx h A h→+--=.(Hint:()()()()()()000000f x h f x h f x h f x f x h f x hhh+--+---=-)解:()()()()00000lim →+---⎛⎫=- ⎪⎝⎭h f x h f x f x h f x A h h ()()()()()000000lim 2→+---⎛⎫'=+= ⎪-⎝⎭h f x hf x f x h f x f x h h3. Find 0()lim sin 2→x f x x, if (0)0f = and (0)2f '=.解:00()()(0)lim lim sin 20sin 2→→-=⋅-xx f x f x f xx x x00()(0)lim lim 0sin 21(0)12→→-=⋅-'=⋅=x x f x fxx x f4. Find derivatives of the following functions by using the derivative formula of power functions:(1)y =解:32==y x ,所以311223322-'==y x x(2)y =; 解:13-==y x,所以141331133---'=-=-y xx(3)3y x =解:1163355+===y x x x,所以1125161655'==y x x5. Take two points with the abscissa 11=x and 33=x on the parabola 2x y =and draw a secant line through the two points. Find the point on the parabola at which the tangent line to the curve is parallel to this secant line.解:当11=x 时,11y =;当23x =时,29y =。
AP考试模拟试题与答案1-微积分BC- AP Calculus-BC
1
14
(A) (B) 1 (C)
(D) 4 (E) 5
2
16
15. Which of the following is an equation of the line tangent to the curve with parametric equations x = 3t2 − 2, y = 2t3 + 2 at the point when t = 1?
−
d 3)
x
=
n
5x
(A) lim n→0
−3
(x
+
2)(x
−
dx 3)
−2
5x
(B) lim n→− 3 + n
(x
+
2)(x
−
dx 3)
n
5x
(C) lim n→− 2−
− 3 (x + 2)(x − 3) d x
n
5x
(D) lim n→− 3
ห้องสมุดไป่ตู้
−3
(x
+
2)(x
−
dx 3)
−2
5x
(E) lim n→0 n
III. f < 0 on (0, b)
(A) III only (B) I and II only
(C) II and III only (D) I and III only (E) I, II, and III
y f
a
0
x b
Figure 1T-3
7.
∞
1
=
n = 1 (2n − 1)(2n + 1)
AP微积分_calculus_BC真题答案-048

0
3
1 1 3 f '(t )dt 1 1 2 2 2 2 2
f (3) f (0)
3 9 2 2
4 1 f (4) f (0) f '(t )dt (8 2 2 ) 8 2 0 2 f (4) f (0) 8 2 5 2
2003 AP calculus BC EXAM 答案
1-5 ECADC 6-10 CCBAC 11-15 DEAEB 16-20 CACDD 21-25 BEBDD 26-28 DED 76-80 EACDA 81-85 AAECA 86-90 CCCDB 91-92 BC
Free-response
dx is not positive because x(t ) is decreasing along the arc BD as t increase. dt
(b)
t t 1 dx =0; cos( ) 0 or sin( )0 dt 6 2
t
6
2
or
t 1
2
; t 3 for both.
QUESTION1 Point of intersection e 3 x x at (T, S) =(0.238734, 0.488604)
(a) Area =
1
T
( x e 3 x )dx 0.442 or 0.443
1
(b) Volume = ((1 e 3 x )2 (1 x )2 )dx 1.423 or 1.424
4.52 2.52 5.147 or 5.148
9
(d) x(9) x (0)
AP考试模拟试题与答案1-微积分BC- AP Calculus-BC

6. The graph of the function f is shown in Figure 1T-3. Which of the following statements is/are true?
I. f (0) = 0
II. f has an absolute maximum value on [a , b]
Use the answer sheet provided on the previous page. All questions are given equal weight. Points are not deducted for incorrect answers and no points are given to unanswered questions. Unless otherwise indicated, the domain of a function f is the set of all real numbers. The use of a calculator is not permitted in this part of the exam.
GO ON TO THE NEXT PAGE
(C) e 2
x
a
0
b
(A)
y
Figure 1T-1 A possible graph of f is (see Figure 1T-2):
(B)
y
(C)
y
a
b
x
a0
b
xa
0
bx
(D)
y
(E)
y
a0
b
x
a
0
bx
Figure 1T-2
微积分试卷-英文版3

⑵d(arctan x) _ 1/(1+x A2) _____ .1(3) — dx -cotx .sin x(4). (e2x)(n)泰勒展开式(书上有。
)26/3(6) The right propositi on in the follow ing propositi ons is A .A. If lim f (x) exists and lim g(x) does not exist then lim( f(x) g(x)) does notx a x a x aexist.B. If lim f (x), lim g(x)do both not exist then lim( f (x) g(x)) does not exist.x a x a x aC. If lim f (x) exists and lim g(x)does not exist then lim f (x)g(x) does notx a x a x aexist.D. If lim f (x) exists and lim g(x)does not exist thenx a x aman Xf(x) does not exist.g(x)(7)The right propositi on in the followi ng propositi ons isA. If lim f (x) f (a)then f (a) exists.x aB. If lim f (x) f (a) then f (a) does not exist.x aC. If f (a) does not exist then lim f (x) f (a).x aD. If f (a) does not exist then the cure y f (x) does not have tangent at (a, f (a)).(8) The right statement in the following statements is __A. lim 叱1x x1 B. lim(1 x),xC. x dx —x 1 C1 D.a1 x5(10) The right propositi on in the followi ng propositi ons is _ ____ DA. If f (x) is discontinuous on [a,b]then f(x)is unbounded on [a,b].B. If f (x) is unbounded on [a,b] then f (x) is discontinuous on [a, b].C. If f (x) is bounded on [a,b] then f (x) is continuous on [a,b].求导为2*x*y(x) (y(x)就是y) 5、Find 严吟 dx . x 2(1 x 2)arcta nx/x A2-arcta nx/(1+x A2)=-(arctanx)/x+ / dx/[x(1+xA2)]=-(arctanx)/x+-xyd ((1+xA2)+ / dx/x=-(arctanx)/x- (1/2) / d(1+xA2)/(1+xA2)+ / dx/x=-(arcta nx)/x-(1/2)l n(1+xA2)+l n|x|+Cexpressi ons is _ C ___“ d bA. (a f(x)dx) f (b) db ad bC. ( f (x)dx) 0 dx aba f(x)dx)b f (x)dx)af (a) f(b) f(a)D. If f (x) has absolute extreme values on [a,b] then f(x) is continuouson [a,b].3、Evaluate 1/24 . Find dy|x °and y (0) if x20 yGt)dte x .隐函数求导。
高三英语微积分基础单选题20题

高三英语微积分基础单选题20题1. In the function f(x) = 3x^2 + 5x - 2, the derivative of f(x) at x = 1 is:A. 11B. 8C. 10D. 12答案:A。
本题考查导数的基本运算。
首先对函数f(x) 求导得到f'(x) = 6x + 5,将x = 1 代入f'(x),得到f'(1) = 6×1 + 5 = 11。
选项B 计算错误;选项C 和D 也是计算错误。
2. If the integral of f(x) from 0 to 2 is 10, and the integral of f(x) from0 to 1 is 4, then the integral of f(x) from 1 to 2 is:A. 6B. 8C. 14D. 16答案:A。
根据定积分的性质,从a 到b 的积分等于从a 到c 的积分加上从 c 到 b 的积分。
所以从1 到 2 的积分为从0 到 2 的积分减去从0 到1 的积分,即10 - 4 = 6。
选项B、C、D 计算错误。
3. The slope of the tangent line to the curve y = x^3 at the point (1, 1) is:A. 1B. 3C. 2D. 4答案:B。
对y = x^3 求导得y' = 3x^2,将x = 1 代入得斜率为3×1^2 = 3。
选项A、C、D 计算错误。
4. The area under the curve y = 2x + 1 from x = 1 to x = 3 is:A. 10B. 12C. 8D. 14答案:A。
先求出定积分,∫(2x + 1)dx = x^2 + x,代入上限3 和下限1,得到(3^2 + 3) - (1^2 + 1) = 12 - 2 = 10。
AP微积分AB 2006 (Form B) 真题解答

AP® Calculus AB2006 Scoring GuidelinesForm BThe College Board: Connecting Students to College SuccessThe College Board is a not-for-profit membership association whose mission is to connect students to college success and opportunity. Founded in 1900, the association is composed of more than 5,000 schools, colleges, universities, and other educational organizations. Each year, the College Board serves seven million students and their parents, 23,000 high schools, and 3,500 colleges through major programs and services in college admissions, guidance, assessment, financial aid, enrollment, and teaching and learning. Among its best-known programs are the SAT®, the PSAT/NMSQT®, and the Advanced Placement Program® (AP®). The College Board is committed to the principles of excellence and equity, and that commitment is embodied in all of its programs, services, activities, and concerns.© 2006 The College Board. All rights reserved. College Board, AP Central, APCD, Advanced Placement Program, AP, AP Vertical Teams, Pre-AP, SAT, and the acorn logo are registered trademarks of the College Board. Admitted Class Evaluation Service, CollegeEd, connect to college success, MyRoad, SAT Professional Development, SAT Readiness Program, and Setting the Cornerstones are trademarks owned by the College Board. PSAT/NMSQT is a registered trademark of the College Board and National Merit Scholarship Corporation. All other products and services may be trademarks of their respective owners. Permission to use copyrighted College Board materials may be requested online at:/inquiry/cbpermit.html.Visit the College Board on the Web: .AP Central is the official online home for the AP Program: .2006 SCORING GUIDELINES (Form B)Question 1Let f be the function given by ()323cos. 432x x xf x x=−−+ Let R be the shaded region in the second quadrant bounded by the graph of f, and let S be the shaded region bounded by the graph of f and line ,l the line tangent to the graph of f at 0,x= as shown above.(a) Find the area of R.(b) Find the volume of the solid generated when R is rotated about thehorizontal line 2.y=−(c) Write, but do not evaluate, an integral expression that can be usedto find the area of S.Let f be the function defined for 0x ≥ with ()05f = and ,f ′ the first derivative of f , given by ()()()42sin .x f x e x −′= The graph of ()y f x ′= is shown above.(a) Use the graph of f ′ to determine whether the graph of f isconcave up, concave down, or neither on the interval 1.7 1.9.x << Explain your reasoning.(b) On the interval 03,x ≤≤ find the value of x at which f hasan absolute maximum. Justify your answer.(c) Write an equation for the line tangent to the graph of f at 2.x =The figure above is the graph of a function of x , which models the height of a skateboard ramp. The function meets the following requirements.(i) At 0,x = the value of the function is 0, and the slope of the graph of the function is 0.(ii) At 4,x = the value of the function is 1, and the slope of the graph of the function is 1.(iii) Between 0x = and 4,x = the function is increasing.(a) Let ()2,f x ax = where a is a nonzero constant. Show that it is not possible to find a value for a so that fmeets requirement (ii) above. (b) Let ()23,16x g x cx =− where c is a nonzero constant. Find the value of c so that g meets requirement (ii) above. Show the work that leads to your answer.(c) Using the function g and your value of c from part (b), show that g does not meet requirement (iii) above.(d) Let (),nx h x = where k is a nonzero constant and n is a positive integer. Find the values of k and n so that h meets requirement (ii) above. Show that h also meets requirements (i) and (iii) above.The rate, in calories per minute, at which a person using an exercise machine burns calories is modeled by the functionf . In the figure above, ()32131f t t t =−++ for04t ≤≤ and f is piecewise linear for 424.t ≤≤ (a) Find ()22.f ′ Indicate units of measure.(b) For the time interval 024,t ≤≤ at what time t is fincreasing at its greatest rate? Show the reasoning that supports your answer.(c) Find the total number of calories burned over the timeinterval 618t ≤≤ minutes.(d) The setting on the machine is now changed so that the person burns ()f t c + calories per minute. For thissetting, find c so that an average of 15 calories per minute is burned during the time interval 618.t ≤≤Consider the differential equation()()21cos .dyy x dxπ=− (a) On the axes provided, sketch a slope field for the given differential equation at the nine points indicated.(Note: Use the axes provided in the exam booklet.)(b) There is a horizontal line with equation y c = that satisfies this differential equation. Find the value of c . (c) Find the particular solution ()y f x = to the differential equation with the initial condition ()10.f =satisfies the differential equation, soA car travels on a straight track. During the time interval 060t ≤≤ seconds, the car’s velocity v , measured infeet per second, and acceleration a , measured in feet per second per second, are continuous functions. The table above shows selected values of these functions. (a) Using appropriate units, explain the meaning of ()6030v t dt ∫in terms of the car’s motion. Approximate()6030v t dt ∫ using a trapezoidal approximation with the three subintervals determined by the table.(b) Using appropriate units, explain the meaning of()30a t dt ∫ in terms of the car’s motion. Find the exact valueof ()300.a t dt ∫(c) For 060,t << must there be a time t when ()5?v t =− Justify your answer. (d) For 060,t << must there be a time t when ()0?a t = Justify your answer.。
ap微积分ab试卷

ap微积分ab试卷English Answer:1. The derivative of f(x) = x^3 + 2x^2 5x + 1 is f'(x)= 3x^2 + 4x 5.2. The integral of f(x) = x^3 + 2x^2 5x + 1 is F(x) = (x^4)/4 + (2x^3)/3 (5x^2)/2 + x + C.3. The limit of (x^2 1)/(x 1) as x approaches 1 is 2.4. The equation of the tangent line to the graph of f(x) = x^3 + 2x^2 5x + 1 at the point (1, -3) is y = 6x 9.5. The area under the curve of f(x) = x^3 + 2x^2 5x + 1 from x = 0 to x = 2 is 9.6. The volume of the solid generated by rotating the region bounded by the curves y = x^2 and y = 4 about the x-axis is (64pi)/5.7. The work done by the force F(x) = x^2 + 2x from x = 0 to x = 2 is 14/3.8. The center of mass of a thin wire of length L with density p(x) = x is at x = L/2.9. The moment of inertia of a thin rod of length L about an axis perpendicular to the rod and passing through one end is (ML^2)/12.10. The Laplace transform of f(t) = e^(-t) is F(s) = 1/(s + 1).中文回答:1. f(x) = x^3 + 2x^2 5x + 1 的导数为 f'(x) = 3x^2 + 4x 5。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
Therefore, the line is a horizontal asymptote
(考点:4.3节,4.5、4.6节。近几年经常会考一道作图题。这种题目应该在注意的点主要包括函数的定义域,对称性,增减区间,极值点,凹凸性,拐点,以及渐近线等。大家参照课本的4.5节进行作图)
(3)Find the intervals of concavity and the inflection points.
Therefore,the concave upwardintervalare , , the concave downwardintervalare , and the inflection points are ,
C.If is bounded on then iscontinuous on .
D.If has absolute extreme values on then iscontinuous on .
3、Evaluate
(考点课本4.4节洛比达法则,每年都会有一道求极限的解答题,大多数都是用洛比达法则去求解,所以大家要注意4.4节的内容。注意洛比达法则的适用范围。)
V=
(考点:求面积以及体积,课本6.1、6.2节。这类题目是常考题,较简单。望同学一定要做相应的题目加以巩固。)
8、Determine the production level that will maximize the profit for a company with cost and demand functions
9、State the second derivative testtheoremtesting maximumand prove it.
Suppose is continuous near c
If and , then has a local maximum atc.
Proof:Because near cand so is concave downward nearc. This means that the graph of liesbelowits horizontal tangent atcand so has a localmaximumatc.
A.If exists and does not existthen does not exist.
B.If , do both not exist then does not exist.
C.If exists and does not existthen does not exist.
D.If exists and does not existthen does not exist.
4.Find and if .
(考察微积分基本定理与微分,书上5.3节)
5、Find =
(凑微分求不定积分,积分是微积分的重点及难点,大家一定要掌握透彻。)
6、Given that .
(1) Find the intervals on which is increasing or decreasing.
and .
Solution
Let since x>0, then x=400
When x=400
Therefore, when the production level is 400 that will maximize the profit 35350
(考点:经济函数,课本4.8节。此题型为常考题,属于送分题,大家可以做相应的4.8节的练习加以巩固)
When
Therefore, the increasinginterval is ,the decreasing interval is
(2) Find the local maximum and minimum values of
The function is increasing in interval ,decreasing in interval ,therefore, the function exist the local minimum value,it is
英文版微积分考试答案
————————————————————————————————作者:
————————————————————————————————日期:
1、(1) 0.
(2)
(3)
(4). .
(5) 26/3
2、
(6)The right proposition in the following propositions is___A_____.
A. B.
C. D.
(10)The right proposition in the following propositions is__B______.
A.If is discontinuous on then is unbounded on .
B.If is unbounded on then isdiscontinuous on .
(8)The right statement in the following statements is___D_____.
AБайду номын сангаас B.
C. D.
(9)For continuous function ,the erroneous expression in the following expressions is____D__.
7、Let R be the region bounded by the curve , and the line and .
(a)Evaluate the area of the region R.
R=
(b)Find the volume of the solid generated by revolving the R about they-axis.
(7)The right proposition in the following propositions is__B______.
A.If then exists.
B.If then does not exist.
C.If does not existthen .
D.If does not existthen the cure does not have tangent at .
