大学物理-保守力与非保守力
大学物理 第三章 动量守恒定律和能量守恒定律 3-5 保守力与非保守力
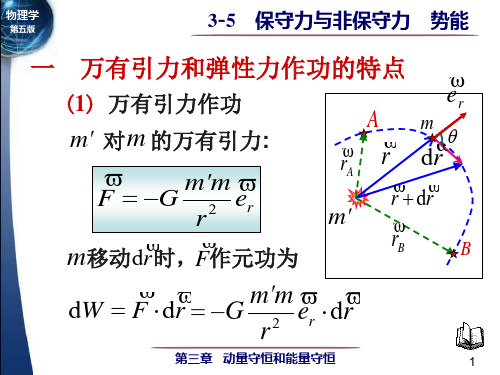
m' m m' m 引力的功 引力的功 WAB = −(−G r ) − (−G r ) B A
A点势能: 点势能: 且令E 设B点为无限远 即rB=∞ 且令 PB=0 点为无限远
m' m WAB = −G rA
= − ( E pB − E pA ) = E pA
功与路径无关,只决定于初末位置。 功与路径无关,只决定于初末位置。 第三章 动量守恒和能量守恒
4
} ⇒ dW
物理学
第五版
3-5 保守力与非保守力 势能 -
F
dW
O
x1
x2
dx
x2 x
W = ∫ Fdx = ∫
x1
x2
x1
1 2 1 2 − kxdx = −( kx2 − kx1 ) 2 2
5
第三章 动量守恒和能量守恒
W p → p0 = −( Ep0 − Ep ) = −∆Ep
E p ( x, y, z) =
∫
E p0 = 0
( x, y,z )
F ⋅ dr
任意一点的势能等于在保守力作用下 从该点到势能零点保守力所作的功
第三章 动量守恒和能量守恒 10
物理学
第五版
3-5 保守力与非保守力 势能 -
W AB = − ( E pB − E pA ) = − ∆ E P
引力的功 引力的功
m' m m' m WAB = −(−G ) − (−G ) rB rA
引力势能 引力势能
m' m Ep = −G r
弹性势能 弹性势能
弹力的功 弹力的功
W AB 1 1 2 2 = − ( kx B − kx A ) 2 2
3-5 保守力和非保守力
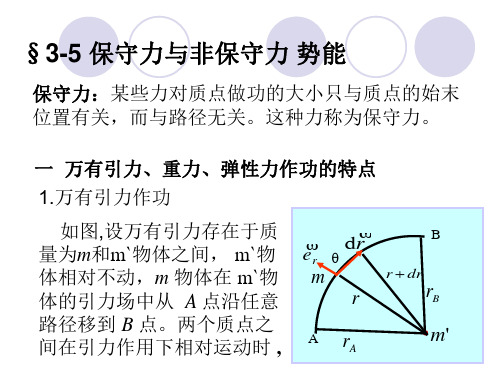
F dr
ADB
F dr
F dr
ADB
W F dr
L
ACB
F dr 0
物体沿闭合路径运动一周时,保守力做功为零
W F dr 0
L
三 势能 E p 1.定义:
设保守力 F 将质点 m 由a→b,保守力的功: b Wab F dr EPa EPb ~势能 E P a
④系统具有势能的条件是物体之间的相互作用力必 须是保守力,而对非保守力系统谈论势能,则没有 任何意义。 如:摩擦力为非保守力,不存在什么摩擦势能。
§3-6 功能原理
机械能守恒定律
动能定理适合于单个物体,也可将其推广到多个 物体组成的系统,成为系统的功能原理。 一、质点系的动能定理 设系统由n个物体(质点)组成,作用于各个质点 的力所作的功分别为:
Mm 1 EP= r -G r 2 dr GMm r
F m r
M
o
③弹性势能
Wab
xb
xa
1 2 1 2 kxdx ( kxb kxa ) EP 2 2
弹性势能以弹簧原长为零势能点。
1 1 2 E P kxdx (0 kx ) kx 2 x 2 2 势能曲线对照表(势能随位置变化的曲线~势能曲线)
重力势能曲线
弹性势能曲线
万有引力势能曲线
WCin ( EPi EPi0 )
i 1 i 1
n
n
质点系的动能定理:
W Wnc ( Eki EPi ) ( Eki0 EPi0 )
ex in i 1 i 1 i 1 i 1
SCUT-3_5保守力与非保守力

m1
v ex Fi
外力功
内力功
v in m i m2 Fi
对质点系,有 对质点系,
∑W
i
ex
i
+ ∑Wi = ∑ Eki − ∑ Eki 0 = Ek − Ek 0
in i i i
质点系动能定理 质点系动能定理
W
ex
+W
in
= Ek − Ek0
第三章 三大守恒定律
值得注意:
内力做功可以改变系统的总动能。 内力做功可以改变系统的总动能。
第三章 三大守恒定律
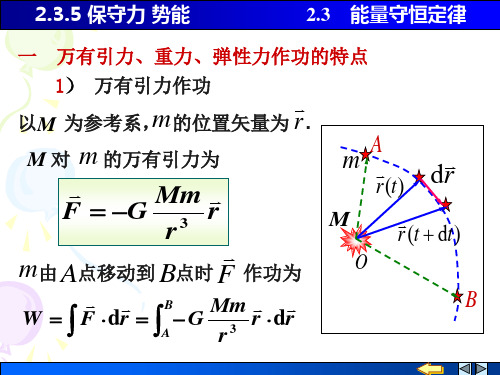
3.5 势能 功能原理 机械能守恒 万有引力、重力、 一 万有引力、重力、弹性力作功的特点 1) 万有引力作功
v m 为参考系, 以 m' 为参考系, 的位置矢量为 r . m' 对 m 的万有引力为
v m' m v F = −G 3 r r v
作功为
m
m'
O
A
v v dr r (t)
m' m Ep = −G r
Ep
O
Ep
x
O
z
O
x
弹性势能曲线 弹性势能曲线 引力势能曲线 引力势能曲线
重力势能曲线 重力势能曲线
z = 0, Ep = 0
x = 0, Ep = 0
r → ∞, Ep = 0
第三章 三大守恒定律
三 质点系的动能定理 个质点, 对第 i 个质点,有
Wi + Wi = E ki − E ki 0
第三章 三大守恒定律
有一轻弹簧, 例 8 有一轻弹簧 其一端系在铅直放置的圆环的 顶点P, 另一端系一质量为m 的小球, 顶点 另一端系一质量为 的小球 小球穿过圆环并 在圆环上运动(不计摩擦 开始小球静止于点 不计摩擦) 在圆环上运动 不计摩擦 .开始小球静止于点 A, 弹簧 处于自然状态,其长度为圆环半径 当小球运动到圆环 处于自然状态 其长度为圆环半径R; 其长度为圆环半径 的底端点B时 小球对圆环没有压力 求弹簧的劲度系数. 小球对圆环没有压力. 的底端点 时,小球对圆环没有压力 求弹簧的劲度系数 解 以弹簧、小球和地球为一系统, 以弹簧、小球和地球为一系统,
保守力与非保守力

保守力与非保守力Document serial number【UU89WT-UU98YT-UU8CB-UUUT-UUT108】非保守力:凡作功与路径有关的力称为非保守力。
常见的摩擦力,物体间相互作非弹性碰撞时的冲击力都属于非保守力。
非保守力具有沿任意闭合路径作功不等于零的特点。
非保守力包括耗散力和非耗散力两类。
在力学范围内接触的非保守力大多数是耗散力,所以长期以来耗散力就成了非保守力的同义词。
严格说来两者是有区别的,一个系统的总机械能减少,并转变为系统的热能或内能。
通常人们把这个过程叫耗散过程,而把导致耗散的力成为耗散力。
摩擦力是耗散力,但非保守力(如爆炸力)不一定都是耗散力。
⑴定义:做功多少只由始末位置所决定,而跟路径无关的力叫做保守力。
做功多少和物体运动路径有关的力叫耗散力。
⑵说明①保守力对物体做功的多少取决于物体始末位置,如果在该力作用下,物体的运动沿闭合路线绕行一周回到了起始位置,则所做功为零。
重力、弹力等属于保守力。
耗散力做功就不能由物体的始末位置决定,而和物体的运动路径有关,在其他条件相同的情况下,物体运动路径越长,所做的功也越多。
摩擦力、粘滞力等属于耗散力②保守力和耗散力所做功的情况不同,是和这两种力的本身的特点有关。
物体系确定后保守力和物体的运动状况无关,其大小由相互作用物体的相对位置所确定,它的方向总在两个相互作用物体的连线上。
例如,物体确定后,重力的大小决定于它离开地面的高度,方向竖直向下,而和物体以什么样的速度运动无关,和物体运动速度的大小和方向如何变化无关。
耗散力的大小和方向都随着物体运动速度的大小、方向的改变而发生变化。
例如,空气对运动物体的阻力,其方向随着物体运动的方向改变而变化,它的大小随物体运动速度增大而增加。
③保守力和物体系的势能有着极为密切的联系。
保守力做正功,则物体系的势能减少;反之,则物体系的势能增加。
而且相对两个位置之间,功量一定,能量差一定。
所以物体间存在保守力是物体系具有势能的条件。
3-5 保守力与非保守力

物理学
第五版
3-5 保守力与非保守力 势能 -
v v v v A F ⋅ dr = ∫ F ⋅ dr ∫ACB ADB v v v v v v ∫l F ⋅ dr = ∫ACB F ⋅ dr + ∫BDA F ⋅ dr v v W = ∫ F ⋅ dr = 0
l
C
第三章 动量守恒和能量守恒 1
物理学
第五版
3-5 保守力与非保守力 势能 -
v m从A到B的过程中 F 作功: 从 到 的过程中 作功:
v v B m'm v v W = ∫ F ⋅ dr = ∫ − G 2 er ⋅ dr A A r v v r v v v v rA er ⋅ dr = er ⋅ dr cos θ = dr
则
v v v v F = Fx i + Fy j + Fz k ∂E P v ∂EP v ∂E P v = − ∂x i + ∂y j + ∂z k
第三章 动量守恒和能量守恒 11
物理学
第五版
3-5 保守力与非保守力 势能 与物体间相互作用及相对位置有关的能量 . 重力势能 重力势能
B
质点沿任意闭合路径运动一周时, 质点沿任意闭合路径运动一周时,保守力 闭合路径运动一周时 对它所作的功为零. 对它所作的功为零. 非保守力:力所作的功与路径有关. 非保守力:力所作的功与路径有关. 例如摩擦 摩擦力 (例如摩擦力)
第三章 动量守恒和能量守恒 6
物理学
第五版
• 势能
3-5 保守力与非保守力 势能 -
势能 重力功 重力功
W = −(mgzB − mgzA )
引力功 引力功 m' m m' m W = −(−G ) − (−G ) rB rA 弹力功 弹力功 引力势能 引力势能
保守力与非保守力
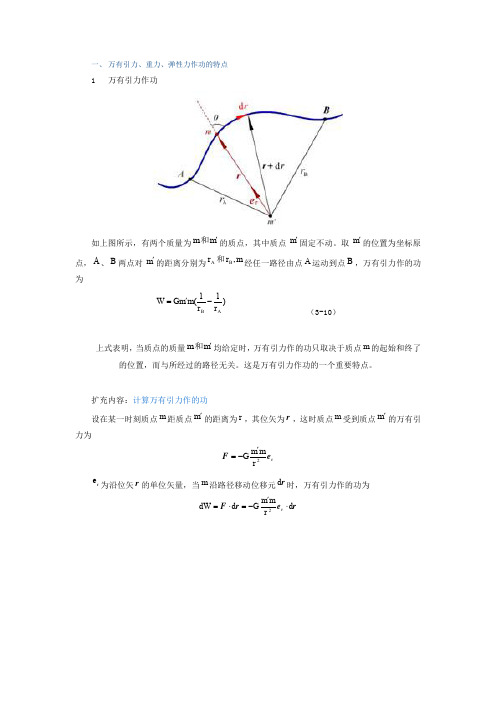
一、 万有引力、重力、弹性力作功的特点1 万有引力作功如上图所示,有两个质量为m m ' 和的质点,其中质点m ' 固定不动。
取m ' 的位置为坐标原点,A 、B 两点对m ' 的距离分别为m r r B A , 和经任一路径由点A 运动到点B ,万有引力作的功为)11(A B r r m m G W -'= (3-10)上式表明,当质点的质量m m ' 和均给定时,万有引力作的功只取决于质点m 的起始和终了的位置,而与所经过的路径无关。
这是万有引力作功的一个重要特点。
扩充内容:计算万有引力作的功设在某一时刻质点m 距质点m '的距离为r ,其位矢为r ,这时质点m 受到质点m '的万有引力为r 2e F r m m G '-=r e 为沿位矢r 的单位矢量,当m 沿路径移动位移元r d 时,万有引力作的功为r e r F d d d r 2⋅'-=⋅=r m m G W从图可以看出r d cos d cos d d r r ===⋅θθr r e r e于是,上式为r r m m G W d d 2'-=所以,质点m 从点A 沿任一路径到达点B 的过程中,万有引力作的功为⎰⎰'-==B A r r B A r r m m G W W 2d 1d即2 重力作功如右图所示,一个质量为m 的质点,在重力作用下从点A 沿ACB 路径至点B ,点A 和点B 距地面的高度分别为21 y y 和,计算重力作功为()12mgy mgy W --= (3-11)上式表明,重力作功只与质点的起始和终了位置有关,而与所经过的路径无关,这是重力作功的一个重要特点。
扩充内容: 计算重力作的功因为质点运动的路径为一曲线,所以重力和质点运动方向之间的夹角是不断变化的。
我们把路径ACB 分成许多位移元,在位移元r d 中,重力P 所作的功为r P d d ⋅=W若质点在平面内运动,按图所选坐标,并取地面上某一点为坐标原点O ,有j i r y x d d d +=且j P mg -=。
保守力与非保守力

非保守力: 凡作功与路径有关的力称为非保守力。
常见的摩擦力,物体间相互作非弹性碰撞时的冲击力都属于非保守力。
非保守力具有沿任意闭合路径作功不等于零的特点。
非保守力包括耗散力和非耗散力两类。
在力学范围内接触的非保守力大多数是耗散力,所以长期以来耗散力就成了非保守力的同义词.严格说来两者是有区别的,一个系统的总机械能减少,并转变为系统的热能或内能。
通常人们把这个过程叫耗散过程,而把导致耗散的力成为耗散力。
摩擦力是耗散力,但非保守力(如爆炸力)不一定都是耗散力。
⑴定义:做功多少只由始末位置所决定,而跟路径无关的力叫做保守力。
做功多少和物体运动路径有关的力叫耗散力。
⑵说明①保守力对物体做功的多少取决于物体始末位置,如果在该力作用下,物体的运动沿闭合路线绕行一周回到了起始位置,则所做功为零.重力、弹力等属于保守力.耗散力做功就不能由物体的始末位置决定,而和物体的运动路径有关,在其他条件相同的情况下,物体运动路径越长,所做的功也越多。
摩擦力、粘滞力等属于耗散力②保守力和耗散力所做功的情况不同,是和这两种力的本身的特点有关。
物体系确定后保守力和物体的运动状况无关,其大小由相互作用物体的相对位置所确定,它的方向总在两个相互作用物体的连线上。
例如,物体确定后,重力的大小决定于它离开地面的高度,方向竖直向下,而和物体以什么样的速度运动无关,和物体运动速度的大小和方向如何变化无关.耗散力的大小和方向都随着物体运动速度的大小、方向的改变而发生变化。
例如,空气对运动物体的阻力,其方向随着物体运动的方向改变而变化,它的大小随物体运动速度增大而增加。
③保守力和物体系的势能有着极为密切的联系.保守力做正功,则物体系的势能减少;反之,则物体系的势能增加。
而且相对两个位置之间,功量一定,能量差一定。
所以物体间存在保守力是物体系具有势能的条件。
系统的各物体在只受保守力作用的情况下其机械能守恒。
耗散力不象保守力,对于两个位置之间,力对物体做功没有确定的值,从而相应的两个位置之间没有一定的能量差。
3–2-保守力与非保守力做功特点-势能知识分享

Ep
G
Mm (R h0 )G NhomakorabeaMm (R
h)
y h
GMm GMm
(R
1
h0 )
(R
1
h )
h h0 ( R h 0 )( R h )
h0 O
R
h , h 0 R
G
Mm R2
mg ( h h 0 )
首页
上页
下页
末页
退出
结论:
重力势能实际上近似等于质点在地面附近两点之 间的引力势能之差,只有当质点在地面附近时,我们 才可以用重力势能代替引力势能计算质点势能的变化 或质点相对于势能零点的势能值,当质点远离地球时, 就必须用质点的引力势能来研究质点的势能了。
物体移动的具体路径无关。
首页
上页
下页
末页
退出
3.万有引力做功
B
M对m的万有引力为
FGM r2 mer
m移动dr时,F 作元功为 M
rB dr rdr
θ
r
m F
d W F d r
rA
GM r2mer dr
A
B Mm
W F d rA Gr2erd r
首页
上页
下页
末页
退出
B Mm
W e r d r F d e r r d A r c Gr2 o ed rr d s r
保守力:力对质点做功而与质点移动的具体路径
无关,而只与质点的初末位置有关。
非保守力: 力所做的功与路
B
径有关。(例如摩擦力)
重力功 W(m2 gm y 1 g ) y D
L C
弹力功
W(1k 2
x2 21 2k1 x2)
§4.2 保守力与非保守力及势能

W保 E p ( E pb E pa ) F保 d r
b a
E 令: pb 0 (即势能零点),则
1. 引入势能条件:
① 质点系。Leabharlann 能属于系统。 ② 保守力作功。Chapter 4. 功和能 作者:杨茂田
Chapter 4. 功和能 作者:杨茂田
§4.2 保守力与非保守力、势能
P.
§4.2 保守力与
非保守力、势能
Chapter 4. 功和能 作者:杨茂田
§4.2 保守力与非保守力、势能
P.
例题1:一马拉雪橇沿着圆弧路面极缓慢匀速运动,设圆 弧路面的半径为R,马队雪橇的拉力总平行于路面,雪橇 的质量为m,与路面的滑动摩擦系数为 ,当把雪橇 由低端拉上45圆弧时,摩擦力和重力对雪橇做功多少? 受力分析得:f 摩擦力做功
Chapter 4. 功和能 作者:杨茂田
§4.2 保守力与非保守力、势能
P.
归纳:
1. 你能证明万有引力作功与路径 1.重力势能: E p ( y ) mgy ( Ep( 0 ) 0 ) 无关吗?
1 2 E ( x ) kx 2. 弹性势能:2. p若选地表为万有引力势能零点,则 ( Ep( 0 ) 0 ) 2 Mm 引力势能表达式如何? ( Ep( ) 0 ) 3. 万有引力势能: p ( r ) G E r
45
N mg cos
Wf
4 f .dr mg cos Rd
0
2 Wf mgR 2
重力做功
2 WG mgh mgR(1 ) 2
Chapter 4. 功和能 作者:杨茂田
大学物理:3_2保守力与非保守力
2.3.5 保守力 势能
2.3 能量守恒定律
2)
重力作功
P mgk
dr dxi dyj dzk
W
B P dr
zB mgdz
A
zA
z
zA
zB
A
mg
B
(mgzB mgz A )
o
x
y
W mgdz 0
2.3.5 保守力 势能
2.3 能量守恒定律
3 ) 弹性力作功
F
A Ek Ek0 Ek
A A外 A内 A外 A非 保 内 A保 内
保守内力的功和势能的关系
A保 内 E p 则有 A外 A非保内 ( E p) Ek
2.3.5 保守力 势能
2.3 能量守恒定律
A外 A非保内 ( E p Ek )
机械能 E Ek Ep
则有 A外 A非 保 内 E
物体 A 和 C, B 和 D 之间摩擦因数均不为零,首 先用外力沿水平方向相向推压 A 和 B, 使弹簧压 缩,后拆除外力, 则 A 和 B 弹开过程中, 对 A、 B、C、D 组成的系统
(A)动量守恒,机械能守恒 . (B)动量不守恒,机械能守恒 . (C)动量不守恒,机械能不守恒 . (D)动量守恒,机械能不一定守恒 .
3、平衡点
dU 0 , 即 : f(x) 0 dx
(G
mr'Am)
重力功 弹力功
W (mgzB mgz A )
W
(
1 2
kxB2
1 2
kxA2
)
A
D
C
F dr F dr
ACB
ADB
B
2.3.5 保守力 势能
2.3 能量守恒定律
《大学物理》3-5-9保守力与非保守力
复习
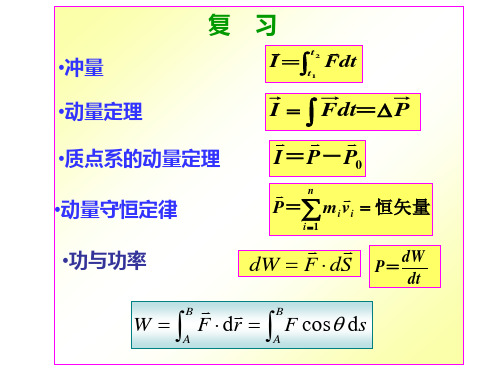
I=
t2
Fdt
t1
•动量定理 •质点系的动量定理
I Fdt= P
I=P-P0
•动量守恒定律 •功与功率
n
P=
mivi
恒矢量
i 1
dW F dS
P= dW
dt
B B
W A F dr A F cos ds
第五次课
保守力与非保守力 ★ 势能
质点系的动能定理 质点系的功能原理
(2) 重力作功
重力mg 在曲线路径 M1M2 上的功为
A
M2
M1 1
Fz
dz
Z2( mg)dz
Z1 1
mg(z1 z2)
z M1
②
m①
M2
G
O
y
x
重力所作的功等于重力的大小乘以质点起始位置与末了
位置的高度差。
结论
(1)重力的功只与始、末位置有关,而与质点所行经的路
径无关。
(2)质点上升时,重力作负功;质点下降时,重力作正功。
Ep
r
(G
mM r2
)dr
等势面
M
mr
G mM
F
r
引力的功
引力势能
W
(G
m' m )
rB
(G
m'm
rA
)
Ep
G
m' m r
弹力的功
弹性势能
W
(
1 2
kxB2
1 2
kx
2 A
)
Ep
1 2
k x2
x
物理学
第五版
3-5 保守力与非保守力 势能
3-4保守力与非保守力

一、万有引力、重力、弹性力作功的特点1 万有引力作功如上图所示,有两个质量为m m ' 和的质点,其中质点m ' 固定不动。
取m ' 的位置为坐标原点,A 、B 两点对m ' 的距离分别为m r r B A , 和经任一路径由点A 运动到点B ,万有引力作的功为)11(A B r r m m G W -'= (3-10)上式表明,当质点的质量m m ' 和均给定时,万有引力作的功只取决于质点m 的起始和终了的位置,而与所经过的路径无关。
这是万有引力作功的一个重要特点。
扩充内容:计算万有引力作的功设在某一时刻质点m 距质点m '的距离为r ,其位矢为r ,这时质点m 受到质点m '的万有引力为r 2e F r m m G '-=r e 为沿位矢r 的单位矢量,当m 沿路径移动位移元r d 时,万有引力作的功为r e r F d d d r 2⋅'-=⋅=rm m G W从图可以看出rd cos d cos d d r r ===⋅θθr re r e 于是,上式为r r m m G W d d 2'-= 所以,质点m 从点A 沿任一路径到达点B 的过程中,万有引力作的功为⎰⎰'-==B A r r B A r r m m G W W 2d 1d 即2 重力作功如右图所示,一个质量为m 的质点,在重力作用下从点A 沿ACB 路径至点B ,点A 和点B 距地面的高度分别为21 y y 和,计算重力作功为()12mgy mgy W --= (3-11)上式表明,重力作功只与质点的起始和终了位置有关,而与所经过的路径无关,这是重力作功的一个重要特点。
扩充内容: 计算重力作的功因为质点运动的路径为一曲线,所以重力和质点运动方向之间的夹角是不断变化的。
我们把路径ACB 分成许多位移元,在位移元r d 中,重力P 所作的功为rP d d ⋅=W若质点在平面内运动,按图所选坐标,并取地面上某一点为坐标原点O ,有ji r y x d d d += 且j P mg -=。
2.3 保守力、非保守力和势能
m'm
dW F dr G r3 r dr
m
A
r (t)
dr
m' r(t dt)
O
B
(3)万有引力作功
说明:r
dr
r
dr
cos
rdr
m'm
m' m
dW F dr G
r3
r dr G
r2
dr
m 由A 点移动到B点时F作功为
W
dw
rB
rA
G
m' m r2
dr
m
A
r (t)
保守力、非保守力 和势能
一、几种常见力作功的特点
(1)弹性力作功
F kxi
dW
F
dx
kxi dxi
o
kxdx
F
x
xA xB
W
dw
xB kxdx
xA
(
1 2
kxB2
1 2
kx
2 A
)
功与路径无关,仅决定于相互作用质点的始末相对位置 .
(2)重力作 功
P mgk
dr
dxi
dyj
dzk
W
B
P
d r
zB mgdz
A
zA
(mgz B mgz A )
z
zA A
zB
mg
B
o
y
x
功与路径无关,仅决定于相互作用质点的始末相对位置 .
(3)万有引力作功
以
m' 为参考系,m
的位置矢量为 r
.
m'对 m 的万有引力为
F
G
m' m r3
4_4保守力与非保守力 势能

三. 保守力
以上通过讨论分析重力、万有引力、弹簧弹性力以及摩擦 力等各种类型力做功的特点,引入保守力与非保守力的概念。
若力所做的功仅仅依赖于受力质点的始末位置, 与质点经过的路径无关,具有这种性质的力称为保 守力。(也可称作有势力). 与保守力相对的称为非保守力:作功与路径有关 的力称为非保守力(nonconservative force) ,或耗散力
对M,内力做功 :
4 – 4
保守力与非保守力 势能
2. 万有引力作功 Work done by universal gravitation B
AAB f dr
rA
rB
dr
m2
r dr
m1
1 1 Gm1 m 2 ( ) rB rA
Gm Gm m 1m 2 2 1 r dr dr 3 rAr r r2 A
rBr
B
r
f
A
A dA AdA
Gm1 m2 f r 3 r
r dr rdr
可见,万有引力作功与路径无关,只与始末位置有关。
4 – 4 A dA
保守力与非保守力 势能
dA
A dA A dA cos
A
r2
dA cos
AdA
4 – 4
保守力与非保守力 势能
一 力 场:
1. 场力:
F F (r )
质点所受的仅与质点位置有关的力.
例如:静电力,弹簧弹性力等.
2. 力场:存在场力的空间 .
例如:存在均匀电场的空间即为均匀力场.
3. 有心力:质点所受力的作用线总通过某一点,则该力
称为有心力(该点称为力心). 例如:万有引力,弹簧弹性力等.
保守力 非保守力 机械能守恒 弹性碰撞
A保内 E p
则有
A外 A非保内 ( E p) E k
2.3.3 功能原理 机械能守恒定律
2.3能量守恒定律
A外 A非保内 ( E p E k )
机械能 则有
E Ek Ep
A外 A非保内 E
质点系的功能原理:质点系机械能的增量等于 外力和非保守内力作功之和 .
2
例5: 一轻弹簧与质量为m1和m2的两 个物体相联结,至少用多大的 力向下压才能在此力撤除后弹 簧把地面的物体带离地面? 解: 设y1、y2、y3分别为弹簧形变量 的绝对值,刚能提起m2的条件:
ky3 m2 g
• 对m1受力分析
ky2 F m1 g
•取坐标原点为零势点,则有
1 2 1 2 ky3 m1 gy3 ky2 m1 gy2 2 2
2 1
2.3.5 保守力 势能
2.3 能量守恒定律
讨论 如图的系统,物体 A,B 置于光滑的桌面上,
物体 A 和 C, B 和 D 之间摩擦因数均不为零,首 先用外力沿水平方向相向推压 A 和 B, 使弹簧压 缩,后拆除外力, 则 A 和 B 弹开过程中, 对 A、 B、C、D 组成的系统 (A)动量守恒,机械能守恒 . (B)动量不守恒,机械能守恒 . (C)动量不守恒,机械能不守恒 . (D)动量守恒,机械能不一定守恒 . C A D B C A D B
引力功 m' m m' m W (G ) (G ) rB rA 弹力功 引力势能
Ep mgz
m' m Ep G r 弹性势能 1 2 Ep kx 2
1 2 1 2 W ( kxB kx A ) 2 2
3-5 保守力和非保守力概述

保守力:某些力对质点做功的大小只与质点的始末 位置有关,而与路径无关。这种力称为保守力。 一 万有引力、重力、弹性力作功的特点 1.万有引力作功 如图,设万有引力存在于质 量为m和m`物体之间, m`物 体相对不动,m 物体在 m`物 体的引力场中从 A 点沿任意 路径移到 B 点。两个质点之 间在引力作用下相对运动时 ,
0
保守力 重 力 弹 力
势能(E p ) mgh
1 2
势能零点 h=0
Ep
0
势能曲线 h
Ep
kx
2
x=0
Ep
0 0
x r
引 力
mM G r
r=∞3.势能和保守力的关系: 势来自是保守力对路径的线积分,EP=
b
a
F dl
F
dEP F dl F cos dl Fdl l
dE P Fl dl
er
dr
r dr
B
m
r
rA
rB
m'
A
以 m 所在处为原点, m 指向 m 的方向为矢径的 正方向。 m 受的引力方向与矢径方向相反。则万 有引力对质点所作的功为:
1 dW F dr Gmm 2 er dr r
er
er dr │ er │ │ dr │ cos │ dr │ cos dr
dW mg dr mgdy
W mgdy
y1 y2
m
y y1 y2
mg
mg ( y2 y1 ) mg ( y1 y2 )
3. 弹性力作功
o 可见,重力是保守力。
如图所示是一放置在光滑平面上的弹簧,弹簧的一 端固定,另一端与一质量为m的物体相连接。
3-5 保守力与非保守力 势能
EP Fx = x
EP Fy = y
EP Fz = z
EP EP EP F = Fx i + Fy j + Fz k = x i + y j + z k
第三章 动量守恒定律和能量守恒定律
v v ∫ F dr = ∫
l
∫
ACB
v v F dr = ∫
ACB
v v F dr + ∫
ADB
v v F dr
BDA
A
C
v v F dr
l 第三章 动量守恒定律和能量守恒定律
v v ∫ F dr = 0
D B
3 – 5 保守力与非保守力 势能
物理学教程 第二版) (第二版)
物体沿闭合路径运动 一周时, 保守力对它所作的 物体沿闭合路径运动 一周时 保守力对它所作的 闭合 功等于零 .
第三章 动量守恒定律和能量守恒定律
3 – 5 保守力与非保守力 势能
物理学教程 第二版) (第二版)
三 势能 势能 与物体间相互作用及相对位置有关的能量 。 重力功 重力势能 重力功 重力势能
W = (mgyB mgyA )
引力功 引力功
E p = mgz
引力势能 引力势能
m m'' m Ep = G r 弹力功 弹性势能 弹力功 弹性势能 1 2 1 2 1 2 E p = kx W = ( kx B kx A ) 保守力的功等于势能增量的负值 2 2 2
v v m移动dr 时, 作元功为 移动 F
m
m'
O
A
v r (t)
v dr
v r (t + dt)
v v m' m v v dW = F dr = G 2 er dr r
保守力和非保守力

4
二、质点在保守力场中的势能
A重 (mgy mgy ) 2 1
1 2 1 2 A弹 ( kx 2 kx1 ) 2 2
Mm Mm A引 [(G ) (G )] r2 r1
保守力作功可以表示为由质点的位置决定的某 种潜在能量(势能)的减少。
1 2 E p ( x) kx c 2
(2)弹性势能
弹性 0 势点一般选在弹簧的原长 x=0 处。则
1 E p (0) k 0 2 c 0 2
c0
6
得到
1 2 E p ( x ) kx 2
Mm c (3)万有引力势能 E p (r ) G r Mm c0 E p () G c 0
保守力
质点在保守力场中的势能
1
一、几种力的功 (1)重力作功 y2 A重 F重 dr mgdy
y1
y 1 o
2
F重 mgj
x
(mgy2 mgy ) 1
z
(2)弹性力作功(原长处为原点)
A弹
x2 F弹 dr kxdx
得到
Mm E p (r ) G r
三、已知保守力场确定势能函数
由 若
b
a
F dr E pa E pb
Epb 0
( 0)
则 E pa a
F dr
7
r 1 2 k Ek mv 2 2r k kr0 k (2) Er r 2 dr r 2 dr r r r
例1、一质量为m 的质点在指向圆心的平方反 比力 F=-k/r2 的作用下作半径为 r 的圆周运动, 求(1)此质点的动能。(2)如取距圆心无穷 远处为势能零点,求它的势能。 2 解(1) F k2 m v r
3–2 保守力与非保守力做功特点 势能

首页
上页
下页
末页
退出
*五、势能曲线
势能曲线:由势能函数确定的势能随坐标变化的曲线。
E p mgy
Ep
Ep
Ep
1 2
kx
2
Ep G
Ep
O
Mm r
r
y
O
O
x
重力势能线
y 0, E p 0
弹性势能曲线
x 0, E p 0
首页
引力势能曲线
r , Ep 0
上页 下页 末页 退出
2
A
m'm m'm W (G ) (G rB rA
F dr
ACB
F dr
)
ADB
首页 上页 下页 末页 退出
F dr
ACB
F dr
ADB
B
F dr
L
F dr
ACB
下页 末页 退出
( x, y,z )
上页
首页
四、重力势能和引力势能的关系
取坐标如图,取y=h0 处为重力势能零点。h处的重 势能为:
Ep G Mm ( R h0 ) G Mm ( R h)
y h h0 O R
1 1 GMm (R h ) ( R h) 0 GMm
利用势能曲线,可以判断物体在各个位置所受保 守力的大小和方向。 当物体受一维保守力作用时
dA dE p
dA F cosdx Fx dx
dE p dx
Fx
保守力沿某坐标轴的分量等于势能对此坐标的导 数的负值。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
4/12
物理学
第五版
3-5 保守力与非保守力 势能 2 保守力作功的数学表达式
∫
(The mathematical expression of work by the conservative force)
ACB
F ⋅ dr = ∫
F ⋅ dr =
ADB
F ⋅ dr
∫
BDA
m 从 A 到 B 的过程中 作功: 的过程中F作功 作功:
A mθ m'm W = ∫ F ⋅ dr = ∫ − G 2 er ⋅ dr dr r A r rA e r dr er ⋅ dr = er ⋅ dr cos θ = dr r + dr
B
rB m'm B W = ∫ − G 2 dr rA r 1 1 m'm W = Gm′m( − ) W = −G dr = 0 2 rB rA l r
Elastic potential energy
m' m Ep = −G r
1 E p = kx 2 2
6/12
第三章 动量守恒和能量守恒
物理学
第五版
3-5 保守力与非保守力 势能 物体在地球表面附近距地面高为y时 具有的引力 物体在地球表面附近距地面高为 时,具有的引力 势能称为重力势能 重力势能(Gravity potenial) Ep = −mgy 重力势能 保守力的功(Work of conservative force) 保守力的功
第三章 动量守恒和能量守恒
z = 0, Ep = 0
11/12
物理学
第五版
本章目录
选择进入下一节: 选择进入下一节:
*3 - 3
系统内质量移动问题
3-4 动能定理 3-5 保守力与非保守力 势能 3-6 功能原理 机械能守恒定律 3-7 完全弹性碰撞 完全非弹性碰撞 3-8 能量守恒定律
第三章 动量守恒和能量守恒
物理学
第五版
3-5 保守力与非保守力 势能
一 万有引力和弹性力作功的特点
(The features of the work done by the universal gravitational & the elastic force) 1、万有引力作功 、
(Work done by universal gravitational force)
12/12
重力势能曲线 重力势能曲线
Curve of gravity potenial energy
引力势能曲线 引力势能曲线
Curve of gravitional potenial energy m'm E p = −G r
E p = mg z
Ep
Ep
O
Ep
x
OБайду номын сангаас
z
O
x
x = 0, Ep = 0 r → ∞, Ep = 0
第三章 动量守恒和能量守恒
7/12
物理学
第五版
3-5 保守力与非保守力 势能
W = − ( E p 2 − E p1 ) = − ∆ E P
在一维情况下
∫
x +∆x
x
F ( x)dx = −∆EP ( x) = −[Ep ( x + ∆x) − Ep ( x)]
作用于物体上的在Ox轴上的保守力 等于势能 作用于物体上的在 轴上的保守力,等于势能 轴上的保守力 对坐标x的导数的负值 的导数的负值。 对坐标 的导数的负值。 The conservative force acting on the object along the Ox axis is equal to the negative derivative of potenial energy with respect to the coordinate x
m’对m的万有引力为 对 的万有引力为
A
rA e r
m'm F = −G er 2 r
m移动 时,F作元功为 移动dr时 作元功为 移动
r
mθ
dr
m'
r + dr
dr
B
m'm dW = F ⋅ dr = −G 2 er ⋅ dr r
第三章 动量守恒和能量守恒
rB
1/12
物理学
第五版
3-5 保守力与非保守力 势能
rB
m'
∫
第三章 动量守恒和能量守恒
2/12
物理学
第五版
3-5 保守力与非保守力 势能 2、弹性力作功 、 (Work done by elastic force)
弹性力
F = − kx i
x2
dW = − kxdx
W = ∫ Fdx = ∫ − kxdx
x1 x1 x2
F
F
P
'
o
F
dW
x
x
1 2 1 2 W = ( kx1 − kx 2 ) 2 2
F ⋅ dr = −∫ F ⋅ dr
ADB
A
C
∫
l
∫
ACB
F ⋅ dr +
∫
BDA
F ⋅ dr
W =
∫
l
F ⋅ dr = 0
D B
质点沿任意闭合路径运动一周时,保守力对它所作的功为零 质点沿任意闭合路径运动一周时 保守力对它所作的功为零 闭合路径运动一周时 保守力对它所作的功为零.
When an object moves around an arbitrary closed loop the work done by conservative forces on it is zero 非保守力:力所作的功与路径有关 如摩擦力) 力所作的功与路径有关(如摩擦力 非保守力 力所作的功与路径有关 如摩擦力 . The non-conservative force:Work done by the force depends on the path(e.g The frictional force)
第三章 动量守恒和能量守恒
5/12
物理学
第五版
3-5 保守力与非保守力 势能
三 势能 (Potential energy)
与相互作用的物体相对位置有关的能量. 与相互作用的物体相对位置有关的能量 Energy depends on of the relative position of the interactive objects 引力的功 引力的功
第三章 动量守恒和能量守恒
∆EP ( x) dEP ( x) F ( x) = − , F ( x) = − ∆x dx
8/12
物理学
第五版
3-5 保守力与非保守力 势能
讨论
势能是状态的函数 势能是状态的函数 状态的
E p = E p ( x, y , z)
The potenial energy is a function of state 势能具有相对性 势能大小与势能零点的选 势能具有相对性,势能大小与势能零点的选 相对性 势能大小与势能零点 有关,任意两点间的势之能差却是绝对的. 任意两点间的势之能差却是绝对的 取有关 任意两点间的势之能差却是绝对的 The relativity of the potenial energy, the value of the potenial energy depends on the selection of the zero point,the difference of the potential energy between any two point has an absolute meaning
Work of gravitional force
弹力的功 弹力的功
of elastic force
Work
m' m m' m 1 2 1 2 W =−[(−G ) −(−G )] W = − ( kx B − kx A ) rB rA 2 2 引力势能 引力势能 弹力势能 弹力势能
Gravitional potential energy
保守力所作的功仅决定于物体始 位置, 保守力所作的功仅决定于物体始、末位置 所作的功仅决定于物体 而与所经过的路径无关. 而与所经过的路径无关. The work done by the conservatiive force only depends on the initial & final positions of the object & is independent of the path that it took.
第三章 动量守恒和能量守恒
9/12
物理学
第五版
3-5 保守力与非保守力 势能
讨论
势能是属于物体系统的 势能是属于物体系统的. 系统的 The potenial energy belong to the system of objects 势能计算(Calculation of potenial energy ) 势能计算
E p1 = 0
E p (x, y, z) =
∫
E p0 = 0
( x,y,z)
F ⋅ dr
10/12
第三章 动量守恒和能量守恒
物理学
第五版
四
3-5 保守力与非保守力 势能 势能曲线(The potenial enery curve) 弹性势能曲线 弹性势能曲线
Curve of elastic potenial energy 1 2 E p = kx 2
W = ∫ −kxdx = 0
l
