2012美赛题目中文翻译
2012年美国数学奥林匹克(USAMO)试题及其解答

2012 年美国数学奥林匹克试题(USAMO)及其解答
田开斌 解答
1、求所有整数 n≥3,使得对于任意 n 个正实数a1 、a2 、a3 、 … … 、an ,如果满足 Max�a1 、a2 、a3 、 … … 、an � ≤ n · Min�a1 、a2 、a3 、 … … 、an �,则其中可以取出三个数, 它们能够构成一个锐角三角形的三条边的长度。 解:对于任意 n≤12,在序列 1、1、√2、√3、√5、√8、√13、√21、√34、√55、√89、12中取前 n 个数,都有 Max�a1 、a2 、a3 、 … … 、an � ≤ n · Min�a1 、a2 、a3 、 … … 、an �,但任意三个数都不能构成 锐角三角形的三条边。所以满足条件的 n≥13。 下面我们用反证法证明 n≥13 时,都满足条件。 我们给 n 个正实数从小到大排序为a1 ≤ a2 ≤ a3 ≤ ⋯ ≤ an ,若其中任意三个数,都不 能构成一个锐角三角形的三条边,则有a1 2 ≤ a2 2 ,ak 2 + ak+1 2 ≤ ak+2 2 ,其中 1≤k≤n-2。 于是知an 2 ≥ fn · a1 2 ,即an ≥ �fn · a1 ,其中fn 为斐波那契数列的第 n 项。又当 n≥13 时, 根据数学归纳法易知都有�fn >n,此时则有an ≥ �fn · a1 >na1 ,与 Max�a1 、a2 、a3 、 … … 、an � ≤ n · Min�a1 、a2 、a3 、 … … 、an �矛盾。所以当 n≥13 时,都 满足条件。 综上所述知,满足条件的 n 为所有不小于 13 的自然数。 2、一个圆被 432 个点等分为 432 段弧,将其中 108 个点染成红色,108 个点染成绿色, 108 个点染成蓝色,108 个点染成黄色。求证:可以在每种颜色的点中各选 3 个点,使得由 同色点构成的四个三角形都全等。 解:我们记 f(m)表示 m 除以 432 的余数,其中 0≤f(m)≤431。 我们从某点开始,按顺时针方向依次给 432 个点排序为 0、1、2、3、……431。设 108 个 红点所在位置依次为a1 、a2 、a3 、 … … 、a108 ,108 个绿点依次为b1 、b2 、b3 、 … … 、b108 , 108 个蓝点依次为c1 、c2 、c3 、 … … 、c108 ,108 个黄点依次为d1 、d2 、d3 、 … … 、d108 。 记Xi = �f(a1 + i)、f(a2 + i)、f(a3 + i)、 … … 、f(a108 + i)� ∩ �b1 、b2 、b3 、 … … 、b108 � b1、b2、b3、……、b108=108(j=1、2、3、……108),所以
2012美赛B题参考资料

Typical Day on the Rogue RiverIf you've never taken a river trip before, it may seem daunting, but read through this description and we think you'll be ready to climb aboard!MorningImagine waking to the smell of coffee brewing or bacon cooking. For the early birds we have coffee, fruit and juice ready by 7 or 7:30 and full breakfast by 8. Breakfast normally consists of something hearty and traditional like pancakes and bacon, french toast and sausage, or scrambled eggs and Canadian bacon. We also have juice, fruit, yogurt and cereal for those who want to eat lighter.Breakfast is a good time to talk about what the day will be like, so people can pack accordingly. For example, if there is a long hike planned, you might put your hiking shoes in your day bag so they will available for the hike.We will have everything packed and ready to hit the river by 9:30 or 10. We don't usually care to be on the river earlier, because it is too cool before then to be getting wet. You will have a chance to read, chat, hike, fish or otherwise relax while the rafts are being loaded.On The WaterRogue River rafting is done in three types of boats.An oar raft is a large raft that is rowed by the guide. Your job here is to relax, watch the scenery go by, and hang on in the rapids.A paddle raft is a bit smaller. Everyone gets a paddle, and works as a team to maneuver the raft. The guide sits in back, steers and give commands.An inflatable kayak, or ducky is a one- or two- person boat. With no guide, it is the most challenging -and fun-way to run the river.Unless you know what kind of boat you want to be in, we recommend that you rotate between boats a few times each day, in order to get the whole river experience.Once on the river we will float for two to three hours before stopping for lunch. We may also stop to take a short hike to a waterfall or other spot of interest.Lunch normally takes about an hour, and gives people another chance to stretch their legs with a short hike or relax.After lunch we float another two to three hours, again perhaps with an off-river activity. We arrive in camp between 3 and 5, depending on the miles we cover and the stops we make.CampWhen we hit camp you will grab your bag and find a nice place to camp. Again, there is time to hike, swim, read, fish, relax or play games. Often a guide will organize a game or a hike to a place of special interest.We serve hors d'oeuvre around 7 and dinner around 8. It is too warm to eat earlier. Dinner is usually a social time when everyone gathers to eat and share their day's experiences. The lead guide usually takes advantage of the gathering to tell everyone what tomorrow will bring.Some folks tend to stay up late, talking, perhaps singing. Instruments are welcome if they are small enough to pack (no pianos, please) and add a great deal to any camp scene. Sometimes, especially on the last night, people put together skits, make up songs, and otherwise celebrate the completion of a wonderful trip together.Dates and PricesTrip ItineraryBefore You Go....Trip Details。
sm1
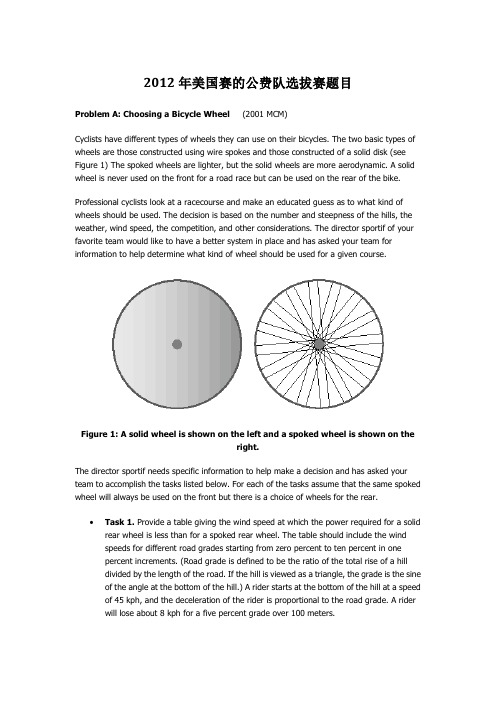
2012年美国赛的公费队选拔赛题目Problem A: Choosing a Bicycle Wheel (2001 MCM)Cyclists have different types of wheels they can use on their bicycles. The two basic types of wheels are those constructed using wire spokes and those constructed of a solid disk (see Figure 1) The spoked wheels are lighter, but the solid wheels are more aerodynamic. A solid wheel is never used on the front for a road race but can be used on the rear of the bike.Professional cyclists look at a racecourse and make an educated guess as to what kind of wheels should be used. The decision is based on the number and steepness of the hills, the weather, wind speed, the competition, and other considerations. The director sportif of your favorite team would like to have a better system in place and has asked your team for information to help determine what kind of wheel should be used for a given course.Figure 1: A solid wheel is shown on the left and a spoked wheel is shown on theright.The director sportif needs specific information to help make a decision and has asked your team to accomplish the tasks listed below. For each of the tasks assume that the same spoked wheel will always be used on the front but there is a choice of wheels for the rear.Task 1. Provide a table giving the wind speed at which the power required for a solid rear wheel is less than for a spoked rear wheel. The table should include the windspeeds for different road grades starting from zero percent to ten percent in onepercent increments. (Road grade is defined to be the ratio of the total rise of a hilldivided by the length of the road. If the hill is viewed as a triangle, the grade is the sine of the angle at the bottom of the hill.) A rider starts at the bottom of the hill at a speed of 45 kph, and the deceleration of the rider is proportional to the road grade. A riderwill lose about 8 kph for a five percent grade over 100 meters.∙Task 2. Provide an example of how the table could be used for a specific time trial course.∙Task 3. Determine if the table is an adequate means for deciding on the wheel configuration and offer other suggestions as to how to make this decision.Problem B: Escaping a Hurricane's Wrath (An Ill Wind...)(2001 MCM)Evacuating the coast of South Carolina ahead of the predicted landfall of Hurricane Floyd in 1999 led to a monumental traffic jam. Traffic slowed to a standstill on Interstate I-26, which is the principal route going inland from Charleston to the relatively safe haven of Columbia in the center of the state. What is normally an easy two-hour drive took up to 18 hours to complete. Many cars simply ran out of gas along the way. Fortunately, Floyd turned north and spared the state this time, but the public outcry is forcing state officials to find ways to avoid a repeat of this traffic nightmare.The principal proposal put forth to deal with this problem is the reversal of traffic on I-26, so that both sides, including the coastal-bound lanes, have traffic headed inland from Charleston to Columbia. Plans to carry this out have been prepared (and posted on the Web) by the South Carolina Emergency Preparedness Division. Traffic reversal on principal roads leading inland from Myrtle Beach and Hilton Head is also planned.A simplified map of South Carolina is shown. Charleston has approximately 500,000 people, Myrtle Beach has about 200,000 people, and another 250,000 people are spread out along the rest of the coastal strip. (More accurate data, if sought, are widely available.)The interstates have two lanes of traffic in each direction except in the metropolitan areas where they have three. Columbia, another metro area of around 500,000 people, does not have sufficient hotel space to accommodate the evacuees (including some coming from farther north by other routes), so some traffic continues outbound on I-26 towards Spartanburg; on I-77 north to Charlotte; and on I-20 east to Atlanta. In 1999, traffic leaving Columbia going northwest was moving only very slowly. Construct a model for the problem to investigate what strategies may reduce the congestion observed in 1999. Here are the questions that need to be addressed:1.Under what conditions does the plan for turning the two coastal-bound lanes of I-26into two lanes of Columbia-bound traffic, essentially turning the entire I-26 intoone-way traffic, significantly improve evacuation traffic flow?2.In 1999, the simultaneous evacuation of the state's entire coastal region was ordered.Would the evacuation traffic flow improve under an alternative strategy that staggers the evacuation, perhaps county-by-county over some time period consistent with the pattern of how hurricanes affect the coast?3.Several smaller highways besides I-26 extend inland from the coast. Under whatconditions would it improve evacuation flow to turn around traffic on these?4.What effect would it have on evacuation flow to establish more temporary shelters inColumbia, to reduce the traffic leaving Columbia?5.In 1999, many families leaving the coast brought along their boats, campers, andmotor homes. Many drove all of their cars. Under what conditions should there berestrictions on vehicle types or numbers of vehicles brought in order to guaranteetimely evacuation?6.It has been suggested that in 1999 some of the coastal residents of Georgia and Florida,who were fleeing the earlier predicted landfalls of Hurricane Floyd to the south, came up I-95 and compounded the traffic problems. How big an impact can they have on the evacuation traffic flow? Clearly identify what measures of performance are used tocompare strategies. Required: Prepare a short newspaper article, not to exceed twopages, explaining the results and conclusions of your study to the public.Clearly identify what measures of performance are used to compare strategies.Required: Prepare a short newspaper article, not to exceed two pages, explaining the results and conclusions of your study to the public.PROBLEM C: Creating Food Systems: Re-Balancing Human-Influenced Ecosystems (2009 ICM, Please see the PDF file “ICM_2009.pdf”)。
美国数学建模题目2001至2012翻译

2001年A题(一)Choosing a Bicycle Wheel选择自行车车轮有不同类型的车轮可以让自行车手们用在自己的自行车上。
两种基本的车轮类型是分别用金属辐条和实体圆盘组装而成(见图1)。
辐条车轮较轻,但实体车轮更符合空气动力学原理。
对于一场公路竞赛,实体车轮从来不会用作自行车的前轮但可以用作后轮。
职业自行车手们审视竞赛路线,并且请一位识文断字的人推断应该使用哪种车轮。
选择决定是根据沿途山丘的数量和陡度,天气,风速,竞赛本身以及其他考虑作出的。
你所喜爱的参赛队的教练希望准备妥当一个较好的系统,并且对于给定的竞赛路线已经向你的参赛队索取有助于确定宜用哪种车轮的信息。
这位教练需要明确的信息来帮助作出决定,而且已经要求你的参赛队完成下面列出的各项任务。
对于每项任务都假定,同样的辐条车轮将总是装在前面,而装在后面的车轮是可以选择的。
任务1. 提供一个给出风速的表格,在这种速度下实体后轮所需要的体能少于辐条后轮。
这个表格应当包括相应于从百分之零到百分之十增量为百分之一的不同公路陡度的风速。
(公路陡度定义为一座山丘的总升高除以公路长度。
如果把山丘看作一个三角形,它的陡度是指山脚处倾角的正弦。
)一位骑手以初始速度45kph从山脚出发,他的减速度与公路陡度成正比。
对于百分之五的陡度,骑上100米车速要下降8kph左右。
任务2. 提供一个例证,说明这个表格怎样用于一条时间试验路线。
任务3. 请判明这个表格是不是一件决定车轮配置的适当工具,并且关于如何作出这个决定提出其他建议。
MCM2001B题Escaping a Hurricane's Wrath逃避飓风怒吼(一场恶风…)1999年,在Floyd飓风预报登陆之前,撤离南卡罗来纳州沿海地区的行动导致一场永垂青史的交通拥塞。
车水马龙停滞在州际公路I-26上,那是内陆上从Charleston通往该州中心Columbia相对安全处所的主要干线。
正常时轻松的两个小时驱车路要用上18个小时才能开到头。
2012年美国大学生数学建模竞赛B题特等奖文章翻译

We develop a model to schedule trips down the Big Long River. The goalComputing Along the Big Long RiverChip JacksonLucas BourneTravis PetersWesternWashington UniversityBellingham,WAAdvisor: Edoh Y. AmiranAbstractis to optimally plan boat trips of varying duration and propulsion so as tomaximize the number of trips over the six-month season.We model the process by which groups travel from campsite to campsite.Subject to the given constraints, our algorithm outputs the optimal dailyschedule for each group on the river. By studying the algorithm’s long-termbehavior, we can compute a maximum number of trips, which we define asthe river’s carrying capacity.We apply our algorithm to a case study of the Grand Canyon, which hasmany attributes in common with the Big Long River.Finally, we examine the carrying capacity’s sensitivity to changes in thedistribution of propulsion methods, distribution of trip duration, and thenumber of campsites on the river.IntroductionWe address scheduling recreational trips down the Big Long River so asto maximize the number of trips. From First Launch to Final Exit (225 mi),participants take either an oar-powered rubber raft or a motorized boat.Trips last between 6 and 18 nights, with participants camping at designatedcampsites along the river. To ensure an authentic wilderness experience,at most one group at a time may occupy a campsite. This constraint limitsthe number of possible trips during the park’s six-month season.We model the situation and then compare our results to rivers withsimilar attributes, thus verifying that our approach yields desirable results.Our model is easily adaptable to find optimal trip schedules for riversof varying length, numbers of campsites, trip durations, and boat speeds.No two groups can occupy the same campsite at the same time.Campsites are distributed uniformly along the river.Trips are scheduled during a six-month period of the year.Group trips range from 6 to 18 nights.Motorized boats travel 8 mph on average.Oar-powered rubber rafts travel 4 mph on average.There are only two types of boats: oar-powered rubber rafts and motorizedTrips begin at First Launch and end at Final Exit, 225 miles downstream.*simulates river-trip scheduling as a function of a distribution of trip*can be applied to real-world rivers with similar attributes (i.e., the Grand*is flexible enough to simulate a wide range of feasible inputs; andWhat is the carrying capacity of the riverÿhe maximum number ofHow many new groups can start a river trip on any given day?How should trips of varying length and propulsion be scheduled toDefining the Problemmaximize the number of trips possible over a six-month season?groups that can be sent down the river during its six-month season?Model OverviewWe design a model thatCanyon);lengths (either 6, 12, or 18 days), a varying distribution of propulsionspeeds, and a varying number of campsites.The model predicts the number of trips over a six-month season. It alsoanswers questions about the carrying capacity of the river, advantageousdistributions of propulsion speeds and trip lengths, how many groups canstart a river trip each day, and how to schedule trips.ConstraintsThe problem specifies the following constraints:boats.AssumptionsWe can prescribe the ratio of oar-powered river rafts to motorized boats that go onto the river each day.There can be problems if too many oar-powered boats are launched with short trip lengths.The duration of a trip is either 12 days or 18 days for oar-powered rafts, and either 6 days or 12 days for motorized boats.This simplification still allows our model to produce meaningful results while letting us compare the effect of varying trip lengths.There can only be one group per campsite per night.This agrees with the desires of the river manager.Each day, a group can only move downstream or remain in its current campsiteÿt cannot move back upstream.This restricts the flow of groups to a single direction, greatly simplifying how we can move groups from campsite to campsite.Groups can travel only between 8 a.m. and 6 p.m., a maximum of 9hours of travel per day (one hour is subtracted for breaks/lunch/etc.).This implies that per day, oar-powered rafts can travel at most 36 miles, and motorized boats at most 72 miles. This assumption allows us to determine which groups can reasonably reach a given campsite.Groups never travel farther than the distance that they can feasibly travelin a single day: 36 miles per day for oar-powered rafts and 72 miles per day for motorized boats.We ignore variables that could influence maximum daily travel distance, such as weather and river conditions.There is no way of accurately including these in the model.Campsites are distributed uniformly so that the distance between campsites is the length of the river divided by the number of campsites.We can thus represent the river as an array of equally-spaced campsites.A group must reach the end of the river on the final day of its trip:A group will not leave the river early even if able to.A group will not have a finish date past the desired trip length.This assumption fits what we believe is an important standard for theriver manager and for the quality of the trips.MethodsWe define some terms and phrases:Open campsite: Acampsite is open if there is no groupcurrently occupying it: Campsite cn is open if no group gi is assigned to cn.Moving to an open campsite: For a group gi, its campsite cn, moving to some other open campsite cm ÿ= cn is equivalent to assigning gi to the new campsite. Since a group can move only downstream, or remain at their current campsite, we must have m ÿ n.Waitlist: The waitlist for a given day is composed of the groups that are not yet on the river but will start their trip on the day when their ranking onthe waitlist and their ability to reach a campsite c includes them in theset Gc of groups that can reach campsite c, and the groups are deemed “the highest priority.” Waitlisted groups are initialized with a current campsite value of c0 (the zeroth campsite), and are assumed to have priority P = 1 until they are moved from the waitlist onto the river.Off the River: We consider the first space off of the river to be the “final campsite” cfinal, and it is always an open campsite (so that any number of groups can be assigned to it. This is consistent with the understanding that any number of groups can move off of the river in a single day.The Farthest Empty CampsiteOurscheduling algorithm uses an array as the data structure to represent the river, with each element of the array being a campsite. The algorithm begins each day by finding the open campsite c that is farthest down the river, then generates a set Gc of all groups that could potentially reach c that night. Thus,Gc = {gi | li +mi . c},where li is the groupÿs current location and mi is the maximum distance that the group can travel in one day.. The requirement that mi + li . c specifies that group gi must be able to reach campsite c in one day.. Gc can consist of groups on the river and groups on the waitlist.. If Gc = ., then we move to the next farthest empty campsite.located upstream, closer to the start of the river. The algorithm always runs from the end of the river up towards the start of the river.. IfGc ÿ= ., then the algorithm attempts tomovethe groupwith the highest priority to campsite c.The scheduling algorithm continues in this fashion until the farthestempty campsite is the zeroth campsite c0. At this point, every group that was able to move on the river that day has been moved to a campsite, and we start the algorithm again to simulate the next day.PriorityOnce a set Gc has been formed for a specific campsite c, the algorithm must decide which group to move to that campsite. The priority Pi is a measure of how far ahead or behind schedule group gi is:. Pi > 1: group gi is behind schedule;. Pi < 1: group gi is ahead of schedule;. Pi = 1: group gi is precisely on schedule.We attempt to move the group with the highest priority into c.Some examples of situations that arise, and how priority is used to resolve them, are outlined in Figures 1 and 2.Priorities and Other ConsiderationsOur algorithm always tries to move the group that is the most behind schedule, to try to ensure that each group is camped on the river for aFigure 1. The scheduling algorithm has found that the farthest open campsite is Campsite 6 and Groups A, B, and C can feasibly reach it. Group B has the highest priority, so we move Group B to Campsite 6.Figure 2. As the scheduling algorithm progresses past Campsite 6, it finds that the next farthest open campsite is Campsite 5. The algorithm has calculated that Groups A and C can feasibly reach it; since PA > PC, Group A is moved to Campsite 5.number of nights equal to its predetermined trip length. However, in someinstances it may not be ideal to move the group with highest priority tothe farthest feasible open campsite. Such is the case if the group with thehighest priority is ahead of schedule (P <1).We provide the following rules for handling group priorities:?If gi is behind schedule, i.e. Pi > 1, then move gi to c, its farthest reachableopen campsite.?If gi is ahead of schedule, i.e. Pi < 1, then calculate diai, the number ofnights that the group has already been on the river times the averagedistance per day that the group should travel to be on schedule. If theresult is greater than or equal (in miles) to the location of campsite c, thenmove gi to c. Doing so amounts to moving gi only in such a way that itis no longer ahead of schedule.?Regardless of Pi, if the chosen c = cfinal, then do not move gi unless ti =di. This feature ensures that giÿ trip will not end before its designatedend date.Theonecasewhere a groupÿ priority is disregardedisshownin Figure 3.Scheduling SimulationWe now demonstrate how our model could be used to schedule rivertrips.In the following example, we assume 50 campsites along the 225-mileriver, and we introduce 4 groups to the river each day. We project the tripFigure 3. The farthest open campsite is the campsite off the river. The algorithm finds that GroupD could move there, but GroupD has tD > dD.that is, GroupD is supposed to be on the river for12 nights but so far has spent only 11.so Group D remains on the river, at some campsite between 171 and 224 inclusive.schedules of the four specific groups that we introduce to the river on day25. We choose a midseason day to demonstrate our modelÿs stability overtime. The characteristics of the four groups are:. g1: motorized, t1 = 6;. g2: oar-powered, t2 = 18;. g3: motorized, t3 = 12;. g4: oar-powered, t4 = 12.Figure 5 shows each groupÿs campsite number and priority value foreach night spent on the river. For instance, the column labeled g2 givescampsite numbers for each of the nights of g2ÿs trip. We find that each giis off the river after spending exactly ti nights camping, and that P ÿ 1as di ÿ ti, showing that as time passes our algorithm attempts to get (andkeep) groups on schedule. Figures 6 and 7 display our results graphically.These findings are consistent with the intention of our method; we see inthis small-scale simulation that our algorithm produces desirable results.Case StudyThe Grand CanyonThe Grand Canyon is an ideal case study for our model, since it sharesmany characteristics with the Big Long River. The Canyonÿs primary riverrafting stretch is 226 miles, it has 235 campsites, and it is open approximatelysix months of the year. It allows tourists to travel by motorized boat or byoar-powered river raft for a maximum of 12 or 18 days, respectively [Jalbertet al. 2006].Using the parameters of the Grand Canyon, we test our model by runninga number of simulations. We alter the number of groups placed on thewater each day, attempting to find the carrying capacity for the river.theFigure 7. Priority values of groups over the course of each trip. Values converge to P = 1 due to the algorithm’s attempt to keep groups on schedule.maximumnumber of possible trips over a six-month season. The main constraintis that each trip must last the group’s planned trip duration. Duringits summer season, the Grand Canyon typically places six new groups onthe water each day [Jalbert et al. 2006], so we use this value for our first simulation.In each simulation, we use an equal number of motorized boatsand oar-powered rafts, along with an equal distribution of trip lengths.Our model predicts the number of groups that make it off the river(completed trips), how many trips arrive past their desired end date (latetrips), and the number of groups that did not make it off the waitlist (totalleft on waitlist). These values change as we vary the number of new groupsplaced on the water each day (groups/day).Table 1 indicates that a maximum of 18 groups can be sent down theriver each day. Over the course of the six-month season, this amounts to nearly 3,000 trips. Increasing groups/day above 18 is likely to cause latetrips (some groups are still on the river when our simulation ends) and long waitlists. In Simulation 1, we send 1,080 groups down river (6 groups/day?80 days) but only 996 groups make it off; the other groups began near the end of the six-month period and did not reach the end of their trip beforethe end of the season. These groups have negligible impact on our results and we ignore them.Sensitivity Analysis of Carrying CapacityManagers of the Big Long River are faced with a similar task to that of the managers of the Grand Canyon. Therefore, by finding an optimal solutionfor the Grand Canyon, we may also have found an optimal solution forthe Big Long River. However, this optimal solution is based on two key assumptions:?Each day, we put approximately the same number of groups onto theriver; and?the river has about one campsite per mile.We can make these assumptions for the Grand Canyon because they are true for the Grand Canyon, but we do not know if they are true for the Big Long River.To deal with these unknowns,wecreate Table 3. Its values are generatedby fixing the number Y of campsites on the river and the ratio R of oarpowered rafts to motorized boats launched each day, and then increasingthe number of trips added to the river each day until the river reaches peak carrying capacity.The peak carrying capacities in Table 3 can be visualized as points ina three-dimensional space, and we can find a best-fit surface that passes (nearly) through the data points. This best-fit surface allows us to estimatethe peak carrying capacity M of the river for interpolated values. Essentially, it givesM as a function of Y and R and shows how sensitiveM is tochanges in Y and/or R. Figure 7 is a contour diagram of this surface.The ridge along the vertical line R = 1 : 1 predicts that for any givenvalue of Y between 100 and 300, the river will have an optimal value ofM when R = 1 : 1. Unfortunately, the formula for this best-fit surface is rather complex, and it doesn’t do an accurate job of extrapolating beyond the data of Table 3; so it is not a particularly useful tool for the peak carrying capacity for other values ofR. The best method to predict the peak carrying capacity is just to use our scheduling algorithm.Sensitivity Analysis of Carrying Capacity re R and DWe have treatedM as a function ofR and Y , but it is still unknown to us how M is affected by the mix of trip durations of groups on the river (D).For example, if we scheduled trips of either 6 or 12 days, how would this affect M? The river managers want to know what mix of trips of varying duration and speed will utilize the river in the best way possible.We use our scheduling algorithm to attempt to answer this question.We fix the number of campsites at 200 and determine the peak carrying capacity for values of R andD. The results of this simulation are displayed in Table 4.Table 4 is intended to address the question of what mix of trip durations and speeds will yield a maximum carrying capacity. For example: If the river managers are currently scheduling trips of length?6, 12, or 18: Capacity could be increased either by increasing R to be closer to 1:1 or by decreasing D to be closer to ? or 12.?12 or 18: Decrease D to be closer to ? or 12.?6 or 12: Increase R to be closer to 4:1.ConclusionThe river managers have asked how many more trips can be added tothe Big Long Riverÿ season. Without knowing the specifics ofhowthe river is currently being managed, we cannot give an exact answer. However, by applying our modelto a study of the GrandCanyon,wefound results which could be extrapolated to the context of the Big Long River. Specifically, the managers of the Big Long River could add approximately (3,000 - X) groups to the rafting season, where X is the current number of trips and 3,000 is the capacity predicted by our scheduling algorithm. Additionally, we modeled how certain variables are related to each other; M, D, R, and Y . River managers could refer to our figures and tables to see how they could change their current values of D, R, and Y to achieve a greater carrying capacity for the Big Long River.We also addressed scheduling campsite placement for groups moving down the Big Long River through an algorithm which uses priority values to move groups downstream in an orderly manner.Limitations and Error AnalysisCarrying Capacity OverestimationOur model has several limitations. It assumes that the capacity of theriver is constrained only by the number of campsites, the trip durations,and the transportation methods. We maximize the river’s carrying capacity, even if this means that nearly every campsite is occupied each night.This may not be ideal, potentially leading to congestion or environmental degradation of the river. Because of this, our model may overestimate the maximum number of trips possible over long periods of time. Environmental ConcernsOur case study of the Grand Canyon is evidence that our model omits variables. We are confident that the Grand Canyon could provide enough campsites for 3,000 trips over a six-month period, as predicted by our algorithm. However, since the actual figure is around 1,000 trips [Jalbert et al.2006], the error is likely due to factors outside of campsite capacity, perhaps environmental concerns.Neglect of River SpeedAnother variable that our model ignores is the speed of the river. Riverspeed increases with the depth and slope of the river channel, makingour assumption of constant maximum daily travel distance impossible [Wikipedia 2012]. When a river experiences high flow, river speeds can double, and entire campsites can end up under water [National Park Service 2008]. Again, the results of our model don’t reflect these issues. ReferencesC.U. Boulder Dept. of Applied Mathematics. n.d. Fitting a surface to scatteredx-y-z data points. /computing/Mathematica/Fit/ .Jalbert, Linda, Lenore Grover-Bullington, and Lori Crystal, et al. 2006. Colorado River management plan. 2006./grca/parkmgmt/upload/CRMPIF_s.pdf .National Park Service. 2008. Grand Canyon National Park. High flowriver permit information. /grca/naturescience/high_flow2008-permit.htm .Sullivan, Steve. 2011. Grand Canyon River Statistics Calendar Year 2010./grca/planyourvisit/upload/Calendar_Year_2010_River_Statistics.pdf .Wikipedia. 2012. River. /wiki/River .Memo to Managers of the Big Long RiverIn response to your questions regarding trip scheduling and river capacity,we are writing to inform you of our findings.Our primary accomplishment is the development of a scheduling algorithm.If implemented at Big Long River, it could advise park rangerson how to optimally schedule trips of varying length and propulsion. Theoptimal schedule will maximize the number of trips possible over the sixmonth season.Our algorithm is flexible, taking a variety of different inputs. Theseinclude the number and availability of campsites, and parameters associatedwith each tour group. Given the necessary inputs, we can output adaily schedule. In essence, our algorithm does this by using the state of theriver from the previous day. Schedules consist of campsite assignments foreach group on the river, as well those waiting to begin their trip. Given knowledge of future waitlists, our algorithm can output schedules monthsin advance, allowing managementto schedule the precise campsite locationof any group on any future date.Sparing you the mathematical details, allow us to say simply that ouralgorithm uses a priority system. It prioritizes groups who are behindschedule by allowing them to move to further campsites, and holds backgroups who are ahead of schedule. In this way, it ensures that all trips willbe completed in precisely the length of time the passenger had planned for.But scheduling is only part of what our algorithm can do. It can alsocompute a maximum number of possible trips over the six-month season.We call this the carrying capacity of the river. If we find we are below ourcarrying capacity, our algorithm can tell us how many more groups wecould be adding to the water each day. Conversely, if we are experiencingriver congestion, we can determine how many fewer groups we should beadding each day to get things running smoothly again.An interesting finding of our algorithm is how the ratio of oar-poweredriver rafts to motorized boats affects the number of trips we can send downstream. When dealing with an even distribution of trip durations (from 6 to18 days), we recommend a 1:1 ratio to maximize the river’s carrying capacity.If the distribution is skewed towards shorter trip durations, then ourmodel predicts that increasing towards a 4:1 ratio will cause the carryingcapacity to increase. If the distribution is skewed the opposite way, towards longer trip durations, then the carrying capacity of the river will always beless than in the previous two cases—so this is not recommended.Our algorithm has been thoroughly tested, and we believe that it isa powerful tool for determining the river’s carrying capacity, optimizing daily schedules, and ensuring that people will be able to complete their trip as planned while enjoying a true wilderness experience.Sincerely yours,Team 13955。
2012美国大学生数学建模题目(英文原版加中文翻译)

2012 MCM ProblemsPROBLEM A:The Leaves of a Tree"How much do the leaves on a tree weigh?" How might one estimate the actual weight of the leaves (or for that matter any other parts of the tree)? How might one classify leaves? Build a mathematical mode l to describe and classify leaves. Consider and answer the following:• Why do leaves have the various shapes that they have?• Do the shapes “minimize” overlapping individual shadows that are cast, so as to maximize exposure? Does the distribution of leaves within the “volume” of the tree and its branches effect the shape?• Speaking of profiles, is leaf shape (general characteristics) related to tree profile/branching structure?• How would you estimate the leaf mass of a tree? Is there a correlation between the leaf mass and the size characteristics of the tree (height, mass, volume defined by the profile)?In addition to your one page summary sheet prepare a one page letter to an editor of a scientific journal outlining your key findings.“多少钱树的叶子有多重?”怎么可能估计的叶子(或树为此事的任何其他部分)的实际重量?会如何分类的叶子吗?建立了一个数学模型来描述和分类的叶子。
2012年河南省翻译竞赛真题

2012年河南省翻译竞赛翻译竞赛英译中参赛原文Over-regulated AmericaThe home of laissez-faire is being suffocated by excessive and badly written regulationAmericans love to laugh at ridiculous regulations. A Florida law requires vending-machine labels to urge the public to file a report if the label is not there. The Federal Railroad Administration insists that all trains must be painted with an “F” at the front, so you can tell which end is which. Bureaucratic busybodies in Bethesda, Maryland, have shut down children’s lemon ade stands because the enterprising young moppets did not have trading licences. The list goes hilariously on.But red tape in America is no laughing matter. The problem is not the rules that are self-evidently absurd. It is the ones that sound reasonable on their own but impose a huge burden collectively. America is meant to be the home of laissez-faire. Unlike Europeans, whose lives have long been circumscribed by meddling governments and diktats from Brussels, Americans are supposed to be free to choose, for better or for worse. Yet for some time America has been straying from this ideal.Consider the Dodd-Frank law of 2010. Its aim was noble: to prevent another financial crisis. Its strategy was sensible, too: improve transparency, stop banks from taking excessive risks, prevent abusive financial practices and end “too big to fail” by authorising regulators to seize any big, tottering financial firm and wind it down. This newspaper supported these goals at the time, and we still do. But Dodd-Frank is far too complex, and becoming more so. At 848 pages, it is 23 times longer than Glass-Steagall, the reform that followed the Wall Street crash of 1929. Worse, every other page demands that regulators fill in further detail. Some of these clarifications are hundreds of pages long. Just one bit, the “Volcker rule”, which aims to curb risky proprietary trading by banks, includes 383 questions that break down into 1,420 subquestions.Hardly anyone has actually read Dodd-Frank. Those who have struggle to make sense of it, not because so much detail has yet to be filled in: of the 400 rules it mandates, only 93 have been finalised. So financial firms in America must prepare to comply with a law that is partly unintelligible and partly unknowable. Flaming water-skisDodd-Frank is part of a wider trend. Governments of both parties keep adding stacks of rules, few of which are ever rescinded. Republicans write rules to thwart terrorists, which make flying in America an ordeal and prompt legions of brainy migrants to move to Canada instead. Democrats write rules to expand the welfare state. Barack Obama’s health-care reform of 2010 had many virtues, especially its attempt to make health insurance universal. But it does little to reduce the system’s staggering and increasing complexity. Every hour spent treating a patient in America creates at least 30 minutes of paperwork, and often a whole hour. Next year the number of federally mandated categories of illness and injury for which hospitals may claim reimbursement will rise from 18,000 to 140,000. There are nine codes relating to injuries caused by parrots, and three relating to burns from flaming water-skis.Two forces make American laws too complex. One is hubris. Many lawmakers seem to believe that they can lay down rules to govern every eventuality. Examples range from the merely annoying (eg, a proposed code for nurseries in Colorado that specifies how many crayons each box must contain) to the delusional (eg, the conceit of Dodd-Frank that you can anticipate and banevery nasty trick financiers will dream up in the future). Far from preventing abuses, complexity creates loopholes that the shrewd can abuse with impunity.The other force that makes American laws complex is lobbying. The government’s drive to micromanage so many activities creates a huge incentive for interest groups to push for special favours. When a bill is hundreds of pages long, it is not hard for congressmen to slip in clauses that benefit their chums and campaign donors. The health-care bill included tons of favours for the pushy. Congress’s last, failed attempt to regulate greenhouse gases was even worse.Complexity costs money. Sarbanes-Oxley, a law aimed at preventing Enron-style frauds, has made it so difficult to list shares on an American stockmarket that firms increasingly look elsewhere or stay private. America’s share of initial public offerings fell from 67% in 2002 (when Sarbox passed) to 16% last year, despite some benign tweaks to the law. A study for the Small Business Administration, a government body, found that regulations in general add $10,585 in costs per employee. It’s a wonder the jobless rate isn’t even higher than it is.A plea for simplicityDemocrats pay lip service to the need to slim the rulebook –Mr Obama’s regulations tsar i s supposed to ensure that new rules are cost-effective. But the administration has a bias towards overstating benefits and underestimating costs (see article). Republicans bluster that they will repeal Obamacare and Dodd-Frank and abolish whole government agencies, but give only a sketchy idea of what should replace them.America needs a smarter approach to regulation. First, all important rules should be subjected to cost-benefit analysis by an independent watchdog. The results should be made public before the rule is enacted. All big regulations should also come with sunset clauses, so that they expire after, say, ten years unless Congress explicitly re-authorises them.More important, rules need to be much simpler. When regulators try to write an all-purpose instruction manual, the truly important dos and don’ts are lost in an ocean of verbiage. Far better to lay down broad goals and prescribe only what is strictly necessary to achieve them. Legislators should pass simple rules, and leave regulators to enforce them.Would this hand too much power to unelected bureaucrats? Not if they are made more accountable. Unreasonable judgments should be subject to swift appeal. Regulators who make bad decisions should be easily sackable. None of this will resolve the inevitable difficulties of regulating a complex modern society. But it would mitigate a real danger: that regulation may crush the life out of America’s economy.选自The Economist, Feb 18th-24th, p8翻译竞赛中译英参赛原文“悦读”的“姿势”从一定意义说,一个民族的发展史就是它的阅读史,一个人亦如此。
2004、2010、2011、2012美国数学建模数学竞赛试题及翻译、分析

2004年A题题目是研究人的指纹相同导致确认身份时产生错误的可能性和因为DNA相同导致产生错误的可能性。
这道题与生物有关。
2004年,美国提出了“科技展望——生物盾国家纳米技术”,针对微分子进行研究,与人体相关的DNA也就成为焦点。
指纹是区分人的重要参数,它由DNA决定,由于现在自然环境改变,人体的DNA也有可能发生变异,这样引起相同指纹出现也是有可能的。
研究这种变异的可能性有利于刑事案件的侦破,提高政府的防御能力等。
本题目可以采用机理分析法来建模。
可以采用计算机模拟人体染色体中的基因排列进而检验、统计排列结果与指纹形成情况B题题目:现在的快通系统在收费站、娱乐公园和其他的地方,正在越来越频繁的使用,来减少人们排队等候的时间,现在我们考虑为一个娱乐公园所设计的快通系统,在一次测试中,这个公园在几个游客比较多的景点旁都设置的快通系统,这个系统的设计创意是对于那些比较热门的景点,可以到旁边的一个机器,将其门票插入后出来一张纸条,上面写着具体的你可以回来时间段,比如说你把你的门票在1:15插到机子里,系统就会告诉你,你可以在3:30-4:30回来,这个时候队伍就比较短,你可以凭你的纸条加入这个队伍,很快就可以进入景点,为了防止游客同时在几个景点使用这个系统。
系统的机器只允许你一次在一个景点排队等候。
现在你是几个被公园雇佣的互相竞争的一个,你的职责是改善快通系统的运行。
很多游客都在抱怨测试期间系统的异常现象,比如说有一次系统提供的回到景点的时间是4小时以后,但是才过一会儿,在相同景点系统提供的时间只有1小时。
在另外一些时候根据快通系统组织起来的游客的等候队伍,就和普通的队伍一样长一样慢。
现在的问题是要提出并且测试一个模型,这个模型能让快通系统的等候纸条的发放能增加人们在公园的乐趣的目的。
问题的一部分就是首先决定衡量不同模型的标准,在你提交的报告里还要附带一份技术性的总结,以便公园的领导在不同的顾问所提出的模型中选择。
美赛题目翻译

美赛题目翻译————————————————————————————————作者:————————————————————————————————日期:2012美赛A题:一棵树的叶子(数学中国翻译)“一棵树的叶子有多重?”怎么能估计树的叶子(或者树的任何其它部分)的实际重量?怎样对叶子进行分类?建立一个数学模型来对叶子进行描述和分类。
模型要考虑和回答下面的问题:•为什么叶子具有各种形状?•叶子之间是要将相互重叠的部分最小化,以便可以最大限度的接触到阳光吗?树叶的分布以及树干和枝杈的体积影响叶子的形状吗?•就轮廓来讲,叶形(一般特征)是和树的轮廓以及分枝结构有关吗?•你将如何估计一棵树的叶子质量?叶子的质量和树的尺寸特征(包括和外形轮廓有关的高度、质量、体积)有联系吗?除了你的一页摘要以外,给科学杂志的编辑写一封信,阐述你的主要发现B:沿着Big Long River野营【数学中国翻译】0 i" k1 T3 h' B# u" ]游客在“大长河”(225英里)可以享受到秀丽的风光和令人兴奋的白色湍流。
这条河对于背包客来说是进不去的,因此畅游这条长河的唯一办法就是在这条河上露营上几天。
这次旅行从开始的下水点到最终结束点,共225英里,且是顺流而下的。
乘客可以选择平均4英里/小时的以浆作为动力的橡胶筏或者平均8英里/小时的机动帆船旅行。
整个旅行从开始到结束会经历6至18个夜晚。
负责管理这条河的政府机构希望到这里的每一次旅行都能够享受到野外经历,以最少的接触到在河上其它的船只。
目前,每年在六个月期间(一年的其余部分的天气对于河流旅行来说太冷),共有X次旅行,有Y 处露营地,露营地均匀的分布整个河道。
由于漂流的受欢迎程度的上升,公园管理者已经被要求允许更多的旅行次数。
所以他们想确定怎样可能安排一个最优的混合的旅行方案,不同的时间(单位为夜)和推动方式(马达或浆),最大限度的利用露营地。
2012 AIME II中文版

2012年美国数学邀请赛(2)1.求满足方程20m+12n=2012的有序正整数解(m,n)的个数.2.两个等比数列a1,a2,a3,⋯和b1,b2,b3,⋯有相同的公比,a1=27,b1=99,并且a15=b11.求a9.3.在一所大学中,数学学院由数学系,统计系和计算机科学系组成.每个系有两位男教授和两位女教授.一个由6名教授组成的委员会包括三位男教授和三位女教授,而且每个系有两位教授.满足上述条件的委员会有多少种可能的组成方式?4.安娜, 鲍勃和曹分别以每秒8.6米,每秒6.2米和每秒5米的均匀速度骑自行车.他们同时从一个矩形区域的东北角出发,矩形区域的长边指向正西方向.安娜出发后起初沿着矩形的边向西前行,鲍勃出发后起先沿着矩形的边向南前行,曹沿着一条直线穿过矩形区域到达矩形南面的边上的一点D.在曹达到D点的同时,安娜和鲍勃也第一次达到D点.矩形的长,宽及D点离矩形东南角的距离之比是p:q:r,这里p,q,r都是正整数且p与q互质,求p+q+r.5.如图所示:外面的正方形S的边长是40,边长为15的第二个正方形S'被构作于S的内部,S'与S有相同的中心,且边互相平行.从S各边中点出发的线段连接S'上与它相邻近的两个顶点,结果得到一个内接于S的四角星图形,剪下此星形图并折叠成一个以S'为底的棱锥,求此棱锥的体积.6. 令复数z=a+bi 有|z|=5,b>0,且使得(1+2i)z 3与z 5之间的距离达到最大,设z 4=c+di,求c+d.7. 令S 是一个递增的正整数数列,其中的每一个数用二进制表达时恰好有8个1,令N 是S 中的第1000个数,求N 被1000除的余数.8. 复数z 和w 满足z+w i 20=5+i,w+z i 12=-4+10i.求|zw|的最小值. 9. 实数x 和y 满足y sin x sin =3,y cos x cos =21,y 2sin x 2sin +y 2cos x 2cos 的值可以写成q p ,这里p 和q 是互质的正整数,求p+q.10. 已知n 是不大于1000的正整数,且存在一个正实数x,使得n=x[x],这样的n 有多少个?(注:[x]表示不大于x 的最大整数)11. 令f 1(x)=32-1x 33+,当n ≥2时,定义f n (x)=f 1(f n-1(x)).满足f 1001(x)=x-3的x 的值可以表示成nm ,这里m 和n 是互质的正整数,求m+n. 12. 对一个正整数p,如果n 与p 的任何一个倍数的差的绝对值都大于2,则定义正整数n 为“p -稳定数”.例如:“10-稳定数”的集合是{3,4,5,6,7,13,14,15,16,17,23,⋯}.求所有不超过10000且同时为“7-稳定数”,“11-稳定数”,“13-稳定数”的正整数的个数.13. 正∆ABC 的边长是111,有四个都与∆ABC 全等的不同的三角形AD 1E 1, AD 1E 2, AD 2E 3和 AD 2E 4,其中BD 1=BD 2=11.求 ∑=41k 2k )CE(.14. 在9个人形成的一个小组中,安排每个人都恰好与小组中的其他两个人握手.N 是可能发生的握手安排方式的种数.两个不同的握手安排,是指当且仅当至少有两个人,他们在某一个安排中握手了,那么他们在另一个安排中不握手.求N被1000除的余数.15.内接于圆ω的∆ABC有AB=5,BC=7,AC=3.∠A的平分线与边BC相交于D,与圆ω的第二个交点是E.设圆γ是以DE为直径的圆,圆ω与m,这里m和n是互质的正整数,求m+n.圆γ的第二个交点是F,AF2=n答案034 363 088 061 750125 032 040 107 496008 958 677 016 919。
历年美国大学生数学建模竞赛试题MCM.(翻译版)doc

1985 年美国大学生数学建模竞赛MCM 试题1985年MCM:动物种群选择合适的鱼类和哺乳动物数据准确模型。
模型动物的自然表达人口水平与环境相互作用的不同群体的环境的重要参数,然后调整账户获取表单模型符合实际的动物提取的方法。
包括任何食物或限制以外的空间限制,得到数据的支持。
考虑所涉及的各种数量的价值,收获数量和人口规模本身,为了设计一个数字量代表的整体价值收获。
找到一个收集政策的人口规模和时间优化的价值收获在很长一段时间。
检查政策优化价值在现实的环境条件。
1985年MCM B:战略储备管理钴、不产生在美国,许多行业至关重要。
(国防占17%的钴生产。
1979年)钴大部分来自非洲中部,一个政治上不稳定的地区。
1946年的战略和关键材料储备法案需要钴储备,将美国政府通过一项为期三年的战争。
建立了库存在1950年代,出售大部分在1970年代初,然后决定在1970年代末建立起来,与8540万磅。
大约一半的库存目标的储备已经在1982年收购了。
建立一个数学模型来管理储备的战略金属钴。
你需要考虑这样的问题:库存应该有多大?以什么速度应该被收购?一个合理的代价是什么金属?你也要考虑这样的问题:什么时候库存应该画下来吗?以什么速度应该是画下来吗?在金属价格是合理出售什么?它应该如何分配?有用的信息在钴政府计划在2500万年需要2500万磅的钴。
美国大约有1亿磅的钴矿床。
生产变得经济可行当价格达到22美元/磅(如发生在1981年)。
要花四年滚动操作,和thsn六百万英镑每年可以生产。
1980年,120万磅的钴回收,总消费的7%。
1986 年美国大学生数学建模竞赛MCM 试题1986年MCM A:水文数据下表给出了Z的水深度尺表面点的直角坐标X,Y在码(14数据点表省略)。
深度测量在退潮。
你的船有一个五英尺的草案。
你应该避免什么地区内的矩形(75200)X(-50、150)?1986年MCM B:Emergency-Facilities位置迄今为止,力拓的乡牧场没有自己的应急设施。
2012年数学建模美赛B题翻译

问题B:大量物种灭绝已经发生
亚马逊雨林是这个世界上最大的雨林,它拥有世界上最多的野生动物。
它座落在南美洲北面被巴西,玻利维亚等9个国家所分享。
因为乱砍乱伐的在雨林造成了致命的影响,所以这条信息需要普及。
虽然短期内砍伐能产生经济效益,但是长期下去会损害亚马逊雨林。
砍伐已经到达了历史的最低点,而在巴西仅仅只有50%的雨林处于被保护的状态。
但是栖息地遗失所产生的影响将在一段时间后会显示出来。
“砍伐在很短的时间内并不能杀死鸟类,长时间就会死亡,它们被挤在狭小的剩下的栖息地里,这样死亡率从就会逐渐提高”,生物学家这样说。
要求:
一.建立砍伐效应的模型,物种大灭绝是否会在未来近期发生。
二.用你的模型区预估多少物种会在未来20年会灭绝,考虑四种情景:
1.商业自由放任砍伐
2.出台一些规定
3.按照政府的规定目标,到2020年为止坚决减少80%的砍伐
4. 2020年终止采伐
三.写一份20页的报告(不包括摘要)表现出你对关于砍伐效应猜想的分析,保证包括政府在确保生态安全方面所扮演的角色。
2012全国大学生英语竞赛决赛B类答案

2012National English Contestfor College Students(Level B-Final)参考答案及评分标准Part I.Listening Comprehension(30points)1—5CBCAB6—10CBBAC11—15BABCA16—20BCACA21.the nguage25.the telegraph 26.steam27.currency28.Europe29.depression30.relationshipsPart II.Multiple-choiceSection A(10points)31—35ABDAC36—40BDCCASection B(5points)41—45DAACDPart III.Cloze(10points)46.fail47.narrow48.distinguishing49.to50.geographic51.products52.account53.secondary54.capture55.strategiesPart IV.Reading comprehension(40points)Section A(10points)56.B57.C58.reproductive system59.enhance performance60.standard doping testsSection B(10points)61.They only got10%of the profits of the band’s merchandising.62.He used the profits to finance his club.63.Because his recording contract greatly favoured the label’s owner.64.He got less than half what the record company had been expecting to pay.65.He rejected a recording company’s offer to appear on the album and film of the music festival Woodstock. Section C(10points)66.most influential writers67.middle class family/Catholic family68.greatest20th-century novel 69.stream of consciousness70.the short-story collectionSection D(10points)71—73YNY1--74.在漆黑的煤窑里我悄悄地、尽可能近地爬到那两个正在说话的人旁边。
美赛题目2010-2018(含目录)

目录2018 年美赛题目翻译 (3)问题A:多跳HF 无线电传播 (3)问题B:语言传播趋势 (3)问题C :能源配置与预测 (5)问题D:从汽油驾驶到E (电)驾驶 (6)问题E:气候变化如何影响区域不稳定? (7)问题F:隐私成本问题 (8)2017 年美赛题目翻译 (10)问题A:管理赞比西河 (10)问题B:收费后合并 (11)问题C:“合作和导航” (12)问题D:在机场安全检查站优化乘客吞吐量 (13)问题E:规划可持续城市的发展 (15)问题F:迁移到火星:2100城市社会的乌托邦劳动力 (17)2016 年美赛题目翻译 (20)Program A 浴缸的水温模型 (20)Program B 解决空间碎片问题 (20)Program C 优质基金挑战 (21)2015 年美赛题目翻译 (21)问题一:根除病毒 (21)问题B:寻找失踪的飞机 (22)2014 年美赛题目翻译 (22)问题A:(交通流、路况)优化 (22)问题B:(体育教练)综合评价 (23)2013 年美赛题目翻译 (23)A :平底锅受热 (23)B:可利用淡水资源的匮乏 (24)2012 年美赛题目翻译 (25)A 题:一棵树的叶子 (25)B:沿着 Big Long River 野营 (25)2011 年美赛题目翻译 (26)A:单板滑雪场地 (26)B:中继站的协调 (26)2010 年美赛题目翻译 (27)A 题:解释棒球棒上的“最佳击球点” (27)B 题系列犯罪地理效应 (27)2018年美赛题目翻译问题A:多跳HF 无线电传播背景:在高频段(HF,定义为3-10MHz),无线电波可以在地球表面和电离层之间的多次反射以进行长距离的传输(从地球表面上的一个点到地球表面上的另一个远点)。
对于低于最大可用频率(MUF)的频率,来自地面源的HF 无线电波将随着每个连续的跳跃继续前进从电离层反射回地球,在那里它们可能再次反射回到电离层,也可能再次反射回地球,等等。
2012年大学生英语竞赛C类阅读题翻译

Section A“文化撞击”这一术语最早出现于1985年,人们用这一术语来描述人在进入全新的环境之后所产生的焦虑。
这种焦虑表现为:处于新环境之中不知道该做什么事,也不知道如何做事。
可以说,“文化撞击”是人们在进入一个不同于以往的新环境时所经历的精神与身体上的不适应的感觉。
通常情况下,新环境中的人们并不接受新成员原来的生活习惯,人们认为那些生活习惯是奇怪的。
所以对于新成员来说,一切都是新的,如:新的语言,不知道怎么使用的取款机,还有不会用的电话,等等。
在很多时候人们都会表现出“文化撞击”的症状。
虽然人们可能由于“文化撞击”而感受到真实的痛楚,但这也是成长和学习的机会,它可以帮助人更好地了解自己,并激发人们的创造力。
“文化撞击”可以分为许多阶段,有些阶段是彼此相连的,而有的阶段则是间隔开来,而且只在特定的时间表现出来。
第一阶段,又叫做“蜜月阶段”。
在这个阶段中,人们初次接触这个新的环境,这种体验让他们觉得很快乐。
随之而来的是第二个阶段,人们有可能会在日常生活中遇到一些困难,例如:交流上的障碍,其他人有可能无法听懂他们的话。
在这个阶段,人们有可能会失去耐心,变得忧伤,愤怒起来。
这个阶段中,人们从旧的生活方式向新的生活方式转变,而这是一个既耗时又费力的过程。
到了第三阶段,人们的一个特点是:他们开始初步了解新环境中的文化了,因此,他们会产生一种愉悦的感觉,还有可能伴随一点幽默感。
从心理上说,人们对新旧环境的感觉趋向于一致,这是因为:人们进一步熟悉了新环境并且开始对新环境产生了归属感。
此外,人们还会将新的生活方式与原来的生活方式加以对比和评价。
在第四阶段,人们认识到新的环境不仅有好的一面也有不好的一面,因此他们会将曾经体验过的两种或者三种文化融合起来。
这种融合的一大特点就是:这个过程中人们有一种强烈的归属感。
人们对开始对自我有了明确的定位,对生活也有了明确的目标。
第五阶段,又叫“二次撞击阶段”,这一阶段是人们回到原来的国家时经历的一个阶段。
2012美赛ICM C题题目翻译

星际犯罪塑型(ICM)正在调查串谋犯犯罪行为。
调查是非常有信心,他们知道的几名成员的阴谋,但他们进行逮捕之前,希望能找出其他成员和领导人。
阴谋和所有可能涉嫌同谋为同一家公司在一个大办公室复杂的工作。
“公司一直快速增长,并为自己的名称,开发和销售计算机银行和信用卡公司的软件。
ICM最近发现了一个消息从一个小集组82个工人,他们认为在公司将帮助他们找到最有可能的候选人身份不明的同谋者和未知的领导人。
由于信息流量是所有的办公在该公司的工人,它很可能是一些(或许很多)在确定的传播者消息流量不涉及阴谋。
事实上,他们是一定的,他们知道有些人谁是不是在阴谋。
建模工作的目标将是确定人们在办公室复杂谁是最有可能的同谋。
一个优先列表将是理想的,因此ICM可以调查,监视之下的地方,和/或询问最有可能的候选人。
一个判别线分离从非同谋的同谋也将是有益的,以明显的分类,在每个人组。
这也将是有用的模型,如果提名的阴谋领导人检察官办公室。
在当前情况下的数据是给你的犯罪建模团队,你的上司给你以下情形(称为调查的EZ),她曾在几年前在另一座城市。
甚至虽然她是她对简易案件的工作感到非常自豪,她说,这是一个非常小的,简单的例子,但它可以帮助你了解自己的任务。
她的数据如下:她考虑为同谋的十人分别为:安妮#,鲍勃,卡罗尔,大卫*,艾伦,弗雷德,乔治·哈利,伊内兹和JAYE#的。
(*表示已知的同谋者,#表示事先已知nonconspirators)28消息,她为她的案件有编号为每个主题年表的消息,她分配的基础上分析她的消息:安妮对鲍勃:你为什么今天迟到了吗?(1)鲍勃对卡罗尔:这该死的安妮总是看着我。
我是不是晚了。
(1)卡罗尔对戴夫:安娜和鲍勃再次战斗是鲍勃的迟到。
(1)戴夫对艾伦:我要看看你今天早晨。
当你能来吗?带来的预算文件。
(2)戴夫对弗雷德:我能来,随时随地今天看到你。
让我知道什么时候是一个好时机。
我应该带来的预算文件吗?(2)戴夫对乔治:我会看到你以后---说不完的话。
美赛历年赛题及其翻译-推荐下载

2015年:A题一个国际性组织声称他们研发出了一种能够阻止埃博拉,并治愈隐性病毒携带者的新药。
建立一个实际、敏捷、有效的模型,不仅考虑到疾病的传播、药物的需求量、可能的给药措施、给药地点、疫苗或药物的生产速度,而且考虑你们队伍认为重要的、作为模型一部分的其他因素,用于优化埃博拉的根除,或至少缓解目前(治疗)的紧张压力。
除了竞赛需要的建模方案以外,为世界医学协会撰写一封1-2页的非技术性的发言稿,以便其公告使用。
B题回顾马航MH370失事事件。
建立一个通用的数学模型,用以帮助失联飞机的搜救者们规划一个有效的搜索方案。
失联飞机从A地飞往B地,可能坠毁在了大片水域(如大西洋、太平洋、印度洋、南印度洋、北冰洋)中。
假设被淹没的飞机无法发出信号。
你们的模型需要考虑到,有很多种不同型号的可选的飞机,并且有很多种搜救飞机,这些搜救飞机通常使用不同的电子设备和传感器。
此外,为航空公司撰写一份1-2页的文件,以便在其公布未来搜救进展的新闻发布会上发表。
2014美赛A题翻译问题一:通勤列车的负载问题在中央车站,经常有许多的联系从大城市到郊区的通勤列车“通勤”线到达。
大多数火车很长(也许10个或更多的汽车长)。
乘客走到出口的距离也很长,有整个火车区域。
每个火车车厢只有两个出口,一个靠近终端, 因此可以携带尽可能多的人。
每个火车车厢有一个中心过道和过道两边的座椅,一边每排有两个座椅,另一边每排有三个座椅。
走出这样一个典型车站,乘客必须先出火车车厢,然后走入楼梯再到下一个级别的出站口。
通常情况下这些列车都非常拥挤,有大量的火车上的乘客试图挤向楼梯,而楼梯可以容纳两列人退出。
大多数通勤列车站台有两个相邻的轨道平台。
在最坏的情况下,如果两个满载的列车同时到达,所有的乘客可能需要很长时间才能到达主站台。
建立一个数学模型来估计旅客退出这种复杂的状况到达出站口路上的时间。
假设一列火车有n个汽车那么长,每个汽车的长度为d。
站台的长度是p,每个楼梯间的楼梯数量是q。
2012年美国国际大学生数学建模竞赛(MCM+ICM)题目+翻译

2012 Contest ProblemsPROBLEM A: The Leaves of a Tree"How much do the leaves on a tree weigh?" How might one estimate the actual weight of the leaves (or for that matter any other parts of the tree)? How might one classify leaves? Build a mathematical model to describe and classify leaves. Consider and answer the following:• Why do leaves have the various shapes that they have?• Do the shapes “minimize” overlapping individual shadows that are cast, so as to maximize exposure? Does the distribution of leaves within the “volume” of the tree and its branches effect the shape?• Speaking of profiles, is leaf shape (general characteristics) related to tree profile/branching structure?• How would you estimate the leaf mass of a tree? Is there a correlation between the leaf mass and the size characteristics of the tree (height, mass, volume defined by the profile)?In addition to your one page summary sheet prepare a one page letter to an editor of a scientific journal outlining your key findings.2012美赛A题:一棵树的叶子(数学中国翻译)“一棵树的叶子有多重?”怎么能估计树的叶子(或者树的任何其它部分)的实际重量?怎样对叶子进行分类?建立一个数学模型来对叶子进行描述和分类。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
Ellen to George:你和Dave谈过了吗?(3)
George to Ellen:还没。你呢?(3)
Bob to Anne:我没有迟到。而且你知道我午饭时间都在工作呢。(1)
Bob to Dave:告诉他们我没有迟到。你了解我的。(1)
Ellen to Carol:联系Anne安排下个星期的预算会议日程,还有,帮我让George冷静点。(2)
她给你看的简单案件的小例子,只有10个人(节点),27条边(消息),5个主题,1个可疑/阴谋主题,2个确定的罪犯,2个已知的清白者。而到目前为止,这个新的案件却已经有83个节点,400条边(有些不止涉及1个主题),超过21000个单词的消息记录,15个主题(其中3个已被视为是可疑的),7个已知的罪犯,和8个已知的清白者。这些数据在所附的电子表格文件:names.xls,Topics.xls,Messages.xls中给出。
3)重要的未知的问题,可能是阴谋,
4)乔治的压力,
5)午餐和其他社会问题。
正如看到的消息编码那样,一些消息根据内容有两个主题。
你的上司按照通信联系和消息类型构造的通信网络分析案件。下图是一个消息网络模型,网络图上注明了消息类型的代码。
您的上司说,除了已知的同谋George and Dave之外,根据她的分析 Ellen and Carol也被认为是同谋。而且不久后,Bob招认出他确实参与其中,从而希望得到减刑。而对Carol的控告后来被放弃了。
A题:
“一棵树的叶子有多重?”怎么能估计树的叶子(或者树的任何其它部分)的实际重量?怎样对叶子进行分类?建立一个数学模型来对叶子进行描述和分类。模型要考虑和回答下面的问题:
? 为什么叶子具有各种形状?
? 叶子之间是要将相互重叠的部分最小化,以便可以最大限度的接触到阳光吗?树叶在树干和枝杈的体积间的分布影响叶子的形状吗?
Inez to George:我今天真的很累。你呢,还好吗? (5)
Jaye to Inez:也不怎么样今天(?)。今天一起去吃午饭怎么样? (5)
Inez to Jaye:幸好一切都很平静。我已经精疲力竭,不能做午饭了今天。抱歉! (5)
George to Dave:现在来见我! (3)
Jaye to Anne:你去吃午饭吗今天? (5)
? 就轮廓来讲,叶形(一般特征)是和树的轮廓以及分枝结构有关吗?
? 你将如何估计一棵树的叶子质量?叶子的质量和树的尺寸特征(包括和外形轮廓有关的高度,给科学杂志的编辑写一封信,阐述你的主要发现。
B题:
游客在“大长河”(225英里)可以享受到秀丽的风光和令人兴奋的白色湍流。这条河对于背包客来说是进不去的, 因此畅游这条长河的唯一办法就是在这条河上露营上几天。这次旅行从开始的下水点到最终结束点,共225英里,且是顺流而下的。乘客可以选择平均4英里/小时的以浆作为动力的橡胶筏或者平均8英里/小时的机动帆船旅行。整个旅行从开始到结束会经历6至18个夜晚。负责管理这条河的政府机构希望到这里的每一次旅行都能够享受到野外经历, 以最少的接触到在河上其它的船只。目前, 每年在六个月期间(一年的其余部分的天气对于河流旅行来说太冷),共有X次旅行,有Y处露营地,露营地均匀的分布整个河道。由于漂流的受欢迎程度的上升,公园管理者已经被要求允许更多的旅行次数。所以他们想确定怎样可能安排一个最优的混合的旅行方案,不同的时间(单位为夜)和推动方式(马达或浆),最大限度的利用露营地。换句话说,在长河的漂流季,将会有多少更多的乘船旅行可以加进来?河流的管理者现在雇佣你,为他们提出最佳排程方式和河流承载能力的建议,记住两个露营者不能在同一时间内占据同一个露营地。除了你的一页摘要,准备一页备忘录,对河流的管理者描述你的主要发现。
Harry to Dave:你有没有注意到George今天看上去又很紧张/有压力?(4)
Dave to George:该死的Harry觉得你很紧张。别让他担心,免得他四处打探。(4)
George to Harry:我只是工作得太晚,家里又有点问题。不用担心,我很好。(4)
Ellen to Harry:我忘了今天的会议了,怎么办?Fred会在那的,而且他比我更了解预算。(2)
在这种情况下,不再像图1那样显示消息的主题。而是在文件Messages.xls里给出主题的数目,并在Topics.xls中给以描述。
要求:
要求1:到目前为止,已知Jean, Alex, Elsie, Paul, Ulf, Yao, and Harvey是罪犯,Darlene, Tran, Jia, Ellin, Gard, Chris, Paige, and Este不是罪犯。可以的消息主题是7,11和13。关于主题更多的信息在Topics.xls里。
topic:
财政----公司股票的价格,去年的盈利,前景,来年的销售预测,流动性资产的分配探讨。
探讨公司的新产品线。此消息流量的部分句子包含西班牙语单词。
办公楼的清洁和维修的抱怨
上周的办公室派对的反响和后续讨论。特别关注的是Alex和Elsie 与Paige之间的大争论。
建筑和计算机数据库安全系统的抱怨。几个人,包括Chris和Este,电脑上网有问题并没法获取他们工作所需要的数据。讨论的共识是,该公司在保护运营安全上已经出现问题了。
C题:
破案模型
您的组织,ICM正在调查一个作案阴谋。调查者非常有信心,因为他们知道阴谋集团的几名成员,但他们希望在进行逮捕之前能找出其他成员和领导人。主谋者和所有可能涉嫌同谋的人都以复杂的关系为同一家公司在一个大办公室工作。 这家公司一直快速增长,并在开发和销售适用于银行和信用卡公司的计算机软件方面打出了自己的名气。 ICM最近从一个82个工人的小集体那儿得知了一个消息,他们认为这个消息能将帮助他们在公司里找到目前身份尚不明确的同谋者和未知的领导人的最有可能的人选。由于信息流通涉及到所有的在该公司工作的工人,所以很可能在这次信息流通中有一些(或许很多)已经确定的传播者实际并不涉及阴谋。事实上,他们确定他们知道一些并不参与阴谋的人。
Dave to Fred:我今天随时随地都可以去见你。让我知道什么时候比较好。我需要带预算文件吗? (2)
Dave to George:我待会见你---有很多需要谈的。我希望其他人都准备好。获得这项权利? 很重要。 (3)
Harry to George:你似乎很紧张。怎么回事?不用担心,我们的预算会好的。 (2)(4)
你的上司至今仍然相当肯定Inez也参与了,但却从未对她立案。
你的上司建议您的团队,确定有罪的当事人,使像Inez的人不漏网,像Carol的人不被诬陷,从而增加ICM的信用,使像Bob的人不再有获得减刑的机会。
现在的案件:
你的上司已经把目前的情况下构造成网络状的数据库,它具有和上面相同的结构,只是范围较大。调查者有一些线索表明,一个阴谋正在挪用公司的资金和使用网上诈骗盗窃在该公司做业务的顾客的信用卡资金。
建模工作的目标是确定在这个复杂的办公室里谁是最有可能的同谋。
一个优先级列表是最理想的,因为ICM可以根据这个来调查,**,和/或询问最有可能的候选人。
一个划分非同谋者与同谋者的分割线也将是有益的,因为可以对每个组里的人进行清楚的分类。
如果能提名阴谋的领导人,那对于检察官办公室也是非常有帮助的。
她对她的案件的28个消息记录按照她的分析 依据主题进行了编号。
Anne to Bob:你今天为什么迟到了? (1)
Bob to Carol:这该死的Anne总是看着我。我并没有迟到。 (1)
Carol to Dave: Anne 和 Bob又再为Bob的迟到吵架了。 (1)
Dave to Ellen:我今天早上要见你。你什么时候能来?把预算文件顺便带过来。 (2)
建立模型和算法,把83个节点按照他是阴谋者的可能性大小排序,并解释你的模型和指标。Jerome, Delores, and Gretchen是该公司的高级经理。如果他们三个人中任何一个涉及阴谋
这将是非常有益的。
要求2:优先列表将有神秘变化,如果有新的信息告知我们说主题1也与阴谋有关,而且克里斯是一个阴谋?(即多了两个线索)
担心和讨论的公司会提升谁到一个空缺的副总裁一职。Paige的升职被一些人主张和反对。一些评论指出,Paige是在公司里迅速上升的有争议的经理。
邀请和围绕晚上在保罗家举行的一次秘密会议进行讨论。在讨论中有好几次提醒人们关于本次会议要谨慎,以确保他们没有在Paige面前讨论这个问题。有些消息用西班牙语,可能是秘密代码。 (此消息主题被认为是阴谋。)
在把当前情况下的数据给你的犯罪建模团队之前,你的上司给你以下情形(称为调查EZ),那是她几年前在另一座城市工作时的案例。她对她在简单案件的工作非常自豪,她说,这是一个非常小的,简单的例子,但它可以帮助你了解自己的任务。
她的数据如下:
她认为是同谋的十人分别为Anne#, Bob, Carol, Dave*, Ellen, Fred, George*, Harry, Inez, and Jaye#.(*表示之前已知的同谋,#表示事先已知的非同谋者)
你有没有使用这些基于文件Topics.xls中的主题描述的功能来提高您的模型?
要求4:你的完整报告将最终提交给检察官办公室,所以一定要详细、明确地说明您的假设和方法,但不能超过20页。您可以包括你的程序作为单独的文件中的附件使你的论文不超过页面限制,但包括这些程序不是必须的。你的上司希望ICM是世界最好的解决白领、高科技的阴谋罪的机构,并希望您的方法有助于解决重要的世界各地的案件,特别是那些消息流量非常大的数据库(可能有数万的信息和数百万的单词)。她特别要求你在论文中讨论 :更深入的网络,语义,消息的文本分析内容是如何帮助你的模型和建议的。
