AP Calculus AB review AP微积分复习提纲
AP Calculus AB review AP微积分复习提纲

AP CALCULUS AB REVIEWChapter 2DifferentiationDefinition of Tangent Line with Slop mIf f is defined on an open interval containing c, and if the limitexists, then the line passing through (c, f(c)) with slope mis the tangent line to the graph of f at the point (c, f(c)). Definition of the Derivative of a FunctionThe Derivative of f at x is given byprovided the limit exists. For all x for which this limit exists, f’ is a function of x.*The Power Rule*The Product Rule***The Chain Rule☺Implicit Differentiation (take the derivative on both sides;derivative of y is y*y’)Chapter 3Applications of Differentiation*Extrema and the first derivative test (minimum: − → + , maximum: + → − , + & − are the sign of f’(x) )*Definition of a Critical NumberLet f be defined at c. If f’(c) = 0 OR IF F IS NOT DIFFERENTIABLE AT C, then c is a critical number of f.*Rolle’s TheoremIf f is differentiable on the open interval (a, b) and f (a) = f (b), then there is at least one number c in (a, b) suchthat f’(c) = 0.*The Mean Value TheoremIf f is continuous on the closed interval [a, b] and differentiable on the open interval (a, b), then there existsa number c in (a, b) such that f’(c) = .*Increasing and decreasing interval of functions (take the first derivative)*Concavity (on the interval which f’’ > 0, concave up)*Second Derivative TestLet f be a function such that f’(c) = 0 and the second derivative of f exists on an open interval containing c.1.If f’’(c) > 0, then f(c) is a minimum2.If f’’(c) < 0, then f(c) is a maximum*Points of Inflection (take second derivative and set it equal to 0, solve the equation to get x and plug x value in originalfunction)*Asymptotes (horizontal and vertical)*Limits at Infinity*Curve Sketching (take first and second derivative, make sureall the characteristics of a function are clear)♫ Optimization Problems*Newton’s Method (used to approximate the zeros of a function,which is tedious and stupid, DO NOT HAVE TO KNOW IF U DO NOTWANT TO SCORE 5)Chapter 4 & 5Integration*Be able to solve a differential equation*Basic Integration Rules1)2)3)4)*Integral of a function is the area under the curve*Riemann Sum (divide interval into a lot of sub-intervals, calculate the area for each sub-interval and summation is theintegral).*Definite integral*The Fundamental Theorem of CalculusIf a function f is continuous on the closed interval [a, b]and F is an anti-derivative of f on the interval [a, b], then.*Definition of the Average Value of a Function on an Interval If f is integrable on the closed interval [a, b], then the average value of f on the interval is.*The second fundamental theorem of calculusIf f is continuous on an open internal I containing a, then,for every x in the interval,.*Integration by Substitution*Integration of Even and Odd Functions1) If f is an even function, then.2) If f is an odd function, then.*The Trapezoidal RuleLet f be continuous on [a, b]. The trapezoidal Rule forapproximating is given byMoreover, a n →∞, the right-hand side approaches.*Simpson’s Rule (n is even)Let f be continuous on [a, b]. Simpson’s Rule forapproximating isMoreover, as n→∞, the right-hand side approaches*Inverse functions(y=f(x), switch y and x, solve for x)*The Derivative of an Inverse FunctionLet f be a function that is differentiable on an intervalI. If f has an inverse function g, then g is differentiableat any x for which f’(g(x))≠0. Moreover,, f’(g(x))≠0.*The Derivative of the Natural Exponential FunctionLet u be a differentiable function of x.1. 2. .*Integration Rules for Exponential FunctionsLet u be a differentiable function of x..♠Derivatives for Bases other than eLet a be a positive real number (a ≠1) and let u be a differentiable function of x.1. 2.♠♠*Derivatives of Inverse Trigonometric FunctionsLet u be a differentiable function of x.*Definition of the Hyperbolic Functions。
2014年AP微积分CALCULUS知识点总结
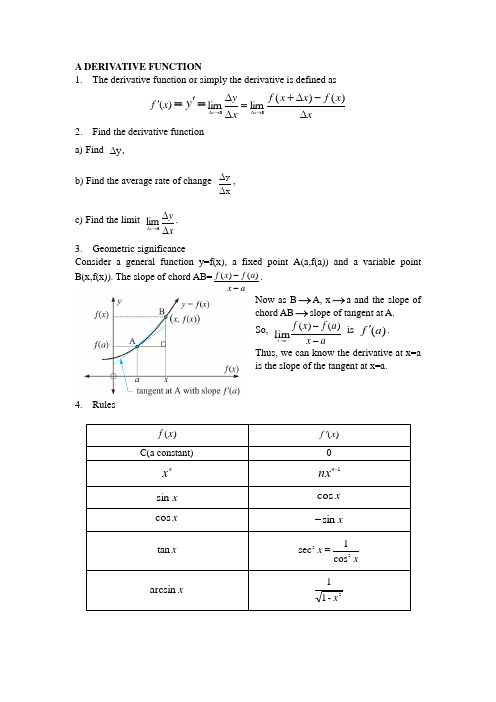
A DERIV ATIVE FUNCTION1. The derivative function or simply the derivative is defined as)(x f '=y '=xx f x x f x y x x ∆-∆+=∆∆→∆→∆)()(lim lim002. Find the derivative function a) Find y ∆,b) Find the average rate of change x y ∆∆, c) Find the limit xy x ∆∆→∆0lim .3. Geometric significanceConsider a general function y=f(x), a fixed point A(a,f(a)) and a variable point B(x,f(x)). The slope of chord AB=ax a f x f --)()(.Now as B →A, x →a and the slope of chord AB →slope of tangent at A. So, ax a f x f a x --→)()(lim is )(a f '.Thus, we can know the derivative at x=a is the slope of the tangent at x=a.4. Rules)(x f)(x f 'C(a constant) 0n x1-n nxx sin x cosx cosx sin -x tanxx 22cos 1sec =x arcsin2-11x5. The chain ruleIf )(u f y = where )(x u u = thendxdu du dy dx dy =. )()(x g e x f = )()()(x g e x f x g '=' )(ln )(x g x f = )()()(x g x g x f '=' )(ln )()(ln )()()()(x u x v x u x v e e x u x f x v ===,])()()()(ln )([)()(ln )(x u x u x v x u x v ex f x u x v '+'='6. Inverse function, Parametric function and Implicit function Inverse function:dy dx dx dy 1=, ])([1)(1'='-x f x f , i.e., x y arcsin =, y x sin =Parametric function:dtdx dtdy dx dy =, i.e., )(t y ϕ=,)(t x ψ=→)(1x t -=ψ, )]([1x y -=ψϕ)()(t t dt dx dt dy dx dt dt dy dx dy ψϕ''=== Implicit function: 0))(,(=x y x F , 0))(,(=x f x F .0-222=+a y x ,ta y t a x sin cos ==, t ]2,0[π∈t ta t a dx dy x y cot sin cos )(-=-=='7. High derivativexx f x x f dx y d x f x ∆'-∆+'==''→∆)()(lim )(022 ta t a t dt dx dt y d dx y d x y x y x 32sin 1sin csc ])([)(-=-='='=''='' xx f x x f x f n n x n ∆-∆+=--→∆)()(lim )()1()1(0)( y=sinx )2sin(cos π+=='x x y , )22sin()2cos(ππ⨯+=+=''x x y )2sin()(π⨯+=n x ynB APPLICATIONS OF DIFFERENTIAL CALCULUS1. Monotonicitya) If S is an interval of real numbers and f(x) is defined for all x in S, then :f(x) is increasing on S ⇔ 0)(≥'x f for all x in S, and f(x) is decreasing on S ⇔0)(≤'x f for all x in S. b) Find the monotone interval ● Find domain of the function,● Find )(x f ', and x which make 0)(='x f , ● Draw sign diagram, find the monotone interval. 2. Maxima/Minima, Horizontal inflection, Stationary pointC INTEGRAL1. The idea of definite integralWe define the unique number between all lower and upper sums as⎰badx x f )( and call it “the definite integral of )(x f from a to b ”,i.e., ∑∑⎰=-=∆〈〈∆ni i n i ba i x x f dx x f x x f 110)()()( where nab x -=∆.We note that as ∞→n ,∑⎰-=→∆10)()(n i ba idx x f x x f and⎰∑→∆=ba ni i dx x f x x f )()(1We write ⎰∑=∆=∞→ba ni i n dx x f x x f )()(lim 1. If 0)(≥x f for all x on [a,b] then⎰badx x f )( is the shaded area.2. Properties of definite integrals⎰⎰-=-bab adx x f dx x f )()]([⎰⎰=ba b a dx x f c dx x cf )()(, c is any constant ⎰⎰⎰=+ca ba cb dx x f dx x f dx x f )()()( ⎰⎰⎰+=+bababadx x g dx x f dx x g x f )()()]()([。
AP-Calculus-AB-review-AP微积分复习提纲

AP-Calculus-AB-review-AP微积分复习提纲AP CALCULUS AB REVIEWChapter 2DifferentiationDefinition of Tangent Line with Slop mIf f is defined on an open interval containing c, and if the limitlim ∆x→0∆y∆x=lim∆x→0f(c+∆x)−f(c)∆x=mexists, then the line passing through (c, f(c)) with slope m is the tangent line to the graph of f at the point (c, f(c)).Definition of the Derivative of a FunctionThe Derivative of f at x is given byf′(x)=lim∆x→0f(c+∆x)−f(c)∆xprovided the limit exists. For all x for which this limit exists, f’ is afunction of x.*The Power Rule*The Product Rule*ddx[sin x]=cos x*ddx[cos x]=−sin x*The Chain Rule☺Implicit Differentiation (take the derivative on both sides;derivative of y is y*y’)Chapter 3Applications of Differentiation*Extrema and the first derivative test (minimum: − → + , maximum:+ → −, + & − are the sign of f’(x) )*Definition of a Critical NumberLet f be defined at c. If f’(c) = 0 OR IF F IS NOTDIFFERENTIABLE AT C, then c is a critical number of f.1)∫u ndu =u n+1n+1+ C,n ≠−12)∫sin u du = −cos u + C 3)∫cos u du = sin u + C 4)∫1u du = ln u*Integral of a function is the area under the curve*Riemann Sum (divide interval into a lot of sub-intervals, calculatethe area for each sub-interval and summation is the integral).*Definite integral*The Fundamental Theorem of CalculusIf a function f is continuous on the closed interval [a, b] and F isan anti-derivative of f on the interval [a, b], then ∫f (x )dx ba=F (b )− F(a).*Definition of the Average Value of a Function on an IntervalIf f is integrable on the closed interval [a, b], then the averagevalue of f on the interval is 1b−a ∫f(x)dx ba.*The second fundamental theorem of calculusIf f is continuous on an open internal I containing a, then, for every x in the interval,ddx[∫f (t )dt xa ]=f(x).*Integration by Substitution*Integration of Even and Odd Functions1) If f is an even function, then ∫f (x )dx b a =2∫f(x)dx ba . 2) If f is an odd function, then ∫f (x )dx ba=0.*The Trapezoidal RuleLet f be continuous on [a, b]. The trapezoidal Rule forapproximating ∫f (x )dx bais given by ∫f (x )dx ba≈ b−a 2n[f (x 0)+2f (x 1)+2f (x 2)+⋯+2f (x n−1) +f (x n )]Moreover, an → ∞, the right-hand sideapproaches ∫f (x )dx ba. *Simpson ’s Rule (n is even)Let f be continuous on [a, b]. Simpson ’s Rule for approximating ∫f (x )dx bais ∫f (x )dx ba≈b −a3n [f (x 0)+4f (x 1)+2f (x 2)+4f (x 3)+⋯4f (x n−1)+f (x n )]Moreover, as n →∞, the right-hand side approaches ∫f (x )dx ba*Inverse functions(y=f (x), switch y and x, solve for x)*The Derivative of an Inverse FunctionLet f be a function that is differentiable on an interval I . If f has aninverse function g , then g is differentiable at any x for which f ’(g (x))≠0. Moreover, g ′(x )= 1f (g(x)), f ’(g (x))≠0.*The Derivative of the Natural Exponential Function Let u be a differentiable function of x .1.d dx[e x ]= e x 2.d dx[e u ]= e udu dx.*Integration Rules for Exponential Functions Let u be a differentiable function of x. ∫e u du = e u +C .♠Derivatives for Bases other than eLet a be a positive real number (a ≠1) and let u be adifferentiable function of x . 1.d dx[a u ]=(ln a)a udu dx2.ddx[log a u ]=1u ln a dudx♠∫a x dx =(1ln a)a x +C♠lim x→∞(1+1x)x =lim x→∞(x+1x)x=e*Derivatives of Inverse Trigonometric Functions Let u be a differentiable function of x. d dx [sin −1u ]=√2d dx[cos −1u ]=√2d dx[tan −1u ]=u′1+u=sin −1u a+C ∫du a 2+u 2=1atan −1ua+C=1a sec −1|u |a+C*Definition of the Hyperbolic Functions sinh x =e x −e −x2 cosh x =e x +e −x2tanh x =sinh x cosh x csch x =1sinh x ,x ≠0 sech x =1cosh x coth x =1tanh x,x ≠0。
(2020年整理)AP Calculus AB review AP微积分复习提纲.pptx

*The Derivative of the Natural Exponential Function Let u be a differentiable fu.
*Integration Rules for Exponential Functions Let u be a differentiable function of x.
*The Mean Value Theorem If f is continuous on the closed interval [a, b] and differentiable on the open interval (a, b), then there exists a number c in (a, b) such that f ’(c) =
area for each sub-interval and summation is the integral). *Definite integral *The Fundamental Theorem of Calculus
If a function f is continuous on the closed interval [a, b] and F is an anti-derivative of f on the interval [a, b], then
*Definition of the Hyperbolic Functions
学海无 涯
exists, then the line passing through (c, f(c)) with slope m is the tangent line to the graph of f at the point (c, f(c)). Definition of the Derivative of a Function The Derivative of f at x is given by
ap微积分大纲英文版

ap微积分大纲英文版The AP Calculus AB and BC course and exam description, published by the College Board, outlines the content and skills students are expected to master in an AP Calculus course. The document provides a detailed breakdown of the topics covered in both the AB and BC courses, as well asthe specific skills and knowledge students need to demonstrate in order to succeed on the AP exam.In the AP Calculus AB course, students are expected to develop a deep understanding of the concepts of limits, derivatives, integrals, and the fundamental theorem of calculus. They also study applications of derivatives and integrals, including but not limited to: analysis of graphs, optimization problems, and modeling with differential equations. Additionally, students are introduced to the concept of series and sequences.The AP Calculus BC course includes all the topics covered in the AB course, but in greater depth and with theaddition of several new topics. These include advanced techniques of integration, parametric, polar, and vector functions, as well as series and Taylor polynomials. The BC course also covers differential equations and the application of calculus to the physical sciences and engineering.In both courses, students are expected to develop a strong foundation in calculus and its applications, including the ability to work with functions represented in a variety of ways: graphical, numerical, analytical, and verbal. They are also expected to develop the skills necessary to justify and interpret results and solutions, and to use calculus to solve problems.The course and exam description provides a comprehensive outline of the content and skills that students need to master, as well as sample exam questions and scoring guidelines. It is an invaluable resource for both teachers and students as they prepare for the AP Calculus exam.。
AP微积分考试的知识点储备

AP微积分考试的知识点储备三立为大家整理了AP微积分考试的知识点储备的相关内容,供考生们参考,以下是详细内容。
1. AP微积分的预备知识AP微积分学习前,学生们应该掌握以下预备知识:(1)实数与数轴(初中知识)(2)绝对值(初中知识)(3)区间和邻域(高中知识)(4)函数的概念(自变量和因变量)、函数表示法(特别是图示法和解析法)、函数的定义域和值域、函数的几何特征:单调性、有界性、奇偶性、周期性。
(高中知识)(5)基本初等函数(常数函数、幂函数、指数函数、对数函数、三角函数和反三角函数)的表达式、定义域和图形。
(高中知识)(6)复合函数对于定义域和值域的理解(高中知识)(7)初等函数和隐函数的表示法和概念(高中知识)(8)数列的基本性质(高中知识)利用高中数学总复习资料可以帮助我们巩固微积分预备知识,国内大学财经类微积分课本的第一章一般会有对高中数学的简单回顾。
SAT1数学部分考的是代数、几何,相当于我国初中知识水平,SAT2数学部分主要包括函数、三角、几何。
SAT2数学分为数学一和数学二,其中数学一比较简单,数学二比较难,包括三角,矩阵,级数,向量和部分微积分。
由于SAT2数学二适用性更广泛,我国学生一般会选考SAT2数学二。
学生可以把准备SAT1数学部分和SAT2数学一和数学二考试的部分内容作为准备学习AP微积分和AP统计学的基础。
AP微积分基础主要在函数和三角。
AP统计学基础主要在概率。
2. AP微积分的学习和考试内容根据最新考试大纲规定的AP微积分的考试内容如下:第一部分:函数和极限(Functions and limits)(1)函数(Functions)(2)函数图像分析(Analysis of graphs)(3)函数的极限(包括单侧极限) (Limits of functions (including one-sided limits)(4)渐进和无穷(Asymptotic and unbounded behavior)(5)函数的连续性(Continuity as a property of functions)第二部分:导数(Derivatives)(1)导数的概念(Concept of the derivative)(2)在一个点处的导数(Derivative at a point)(3)导函数(包括中值定理等) (Derivative as a function)(4)二阶导数(Second derivatives)(5)导数的应用(Applications of derivatives)(6) 导数的运算(Computation of derivatives)第三部分:积分(Integrals)(1)定积分的概念和性质(Interpretations and properties of definite integrals)(2)积分的应用(Applications of integrals)(3)微积分基本定理(Fundamental Theorem of Calculus)(4)不定积分(Techniques of Antidifferentiation)(5)不定积分的应用( Applications of Antidifferentiation)(6)定积分的数值计算( Numerical approximations to definite integrals)第四部分:多项式估算和级数(Polynomial Approximations and Series)(1) 级数的定义(Concept of series)(2) 常数项级数(Series of constant terms)(3) 泰勒级数(Taylor series)注:微积分AB需要1年的课程学习时间,其内容大约占了美国大学一年的微积分课程内容的三分之二,而微积分BC需要1年多的课程学习时间,其内容包括了美国大学一年的微积分课程内容的全部。
ap课程预备微积分大纲

ap课程预备微积分大纲 AP课程预备微积分大纲
I. 概述
A. 课程目标
B. 课程要求
C. 考试信息
II. 函数与图像
A. 函数的定义与性质
B. 常见函数及其图像
C. 函数的变换与组合
III. 极限与连续性
A. 极限的概念与性质
B. 极限计算方法
C. 连续函数与间断点
IV. 导数与微分
A. 导数的定义与性质
B. 导数的计算方法
C. 微分的概念与应用
V. 微分应用
A. 最值与最优化
B. 函数的增减性与凹凸性
C. 泰勒级数与近似计算
VI. 积分与反导数
A. 定积分的定义与性质
B. 积分计算方法
C. 反导数与不定积分
VII. 积分应用
A. 曲线长度与曲面积
B. 微积分与物理学
C. 微积分与经济学
VIII. 微分方程
A. 常微分方程的概念与分类
B. 一阶微分方程的解法
C. 高阶微分方程的解法IX. 多元微积分
A. 多元函数的极限与连续性
B. 偏导数与方向导数
C. 多元函数的积分
X. 空间解析几何
A. 三维空间的坐标系与向量
B. 曲线与曲面方程
C. 空间曲线与曲面的参数化
以上是AP课程预备微积分的大纲,旨在为学生提供必要的基础知识和技能,以便更好地准备和应对AP微积分课程和考试。
大纲包括了函数与图像、极限与连续性、导数与微分、微分应用、积分与反导数、积分应用、微分方程、多元微积分和空间解析几何等内容。
通过系统性的学习和练习,学生将能够掌握微积分的基本概念、计算方法和应用技巧,为未来的学习打下坚实的基础。
ap微积分知识点

ap微积分知识点
AP微积分是高中阶段的一门课程,主要介绍微积分的基本概念和应用。
以下是一些AP微积分的知识点:
1. 导数:导数是函数在某一点的变化率,也可以理解为函数曲线在该点的切线斜率。
常见的导数计算法则包括求常数函数、幂函数、指数函数、对数函数、三角函数等的导数。
2. 微分:微分是导数的另一种表达方式,表示函数在某一点附近的近似线性变化量。
微分可以帮助我们研究函数的极值、曲线的凹凸性等性质。
3. 积分:积分是导数的逆运算,表示函数的累积效应。
通过积分可以计算曲线下的面积、变化量等。
常见的积分计算方法包括不定积分和定积分。
4. 不定积分:不定积分是求导的逆运算,表示函数的原函数。
不定积分的结果通常有一个常数项。
5. 定积分:定积分是计算函数在给定区间上的累积效应,表示曲线下的面积。
定积分可以通过反向求导的方式来计算。
6. 牛顿-莱布尼茨公式:牛顿-莱布尼茨公式是微积分的基本定理之一,它将积分和导数联系在一起。
该公式表明,函数的原函数与其在某一区间上的定积分之间存在关系。
7. 泰勒级数:泰勒级数是一种将函数展开成无穷级数的方法,可以用来近似表示复杂函数。
通过泰勒级数展开,我们可以研究函数的性质和计算函数的近似值。
以上是AP微积分的一些基本知识点,它们构成了微积分的核心内容。
掌握这些知识点能够帮助我们理解函数的变化规律、求解问题以及应用到实际生活中的各种情境中。
ap预备微积分考试内容

ap预备微积分考试内容AP预备微积分考试内容一、微积分初级(Calculus I):1、函数:定义、性质、图形、单调性、增减性等2、导数:概念、直线导数、泰勒级数、泰勒展开、函数的完整导数、极限及极限的性质、微分、求导、导数的应用、高阶导数等3、曲线:曲线的性质、曲线求积、积分、定积分、定积分的性质、变积分、变积分的性质、分部积分、分部积分性质、平均函数等4、圆锥曲线:概念、图形、参数方程、圆锥曲线的长度、圆锥曲线的积分等5、椭圆和抛物线:概念、图形、参数方程、椭圆和抛物线的长度、椭圆和抛物线的积分等6、偏微分:概念、偏微分的概念、偏微分的定义、链式法则、均值值定理等7、多元函数:概念、多元函数的性质、格林函数、极值、偏导数、梯度、拐点、方向梯度、拉格朗日乘子法等二、微积分中级(Calculus II):1、微分方程:概念、一阶微分方程、二阶常系数微分方程、二阶非常系数微分方程、常微分方程的拓展等2、矩阵:概念、矩阵的性质、矩阵的代数和运算、矩阵的行列式、特征值、特征向量、乘积、逆矩阵、线性方程求解等3、空间解析几何:概念、直线、空间抛物线、平面曲线、空间曲线、矢量、矢量求和、向量积、向量场、余弦定理、二次曲面等4、向量函数:概念、函数的几何意义、几何性质、定义域、限域、曲线积分、曲面积分、函数的积分、曲线和曲面的曲率等5、三角函数:概念、三角函数的定义、值域和偏导数、三角法计算面积、余弦定理及解三角形、变换公式和双曲函数等三、微积分高级(Calculus III):1、调和级数:定义、特点、计算和求和、判断收敛性、各种性质等2、积分计算:积分计算技巧、分部积分、不定积分、应用性积分、变量变换、坐标转换、参数形式积分、投影形式积分等3、概率:概率分布、随机变量、极限定理、期望、方差、独立性、随机变量分布、常见概率分布等4、复变函数:定义、初等函数和指数函数、复数的概念和运算、函数的微分和积分、复数的极限和变换、复数函数和应用等5、拓扑学:概念、空间的分类、连续性定理、极限、函数的极限、Countability、连续函数、定理和保守性、定理的应用、复环论等。
ap微积分ab和bc中文讲义

AP微积分AB和BC是大学预修课程,主要涉及微积分的基础知识。
以下是它们的中文讲义:一、AP微积分AB1. 极限与连续极限是研究函数在某一点附近的行为,分为数列极限和函数极限。
连续是指函数在某一点的极限存在且等于该点的函数值。
2. 导数导数表示函数在某一点的切线斜率,反映了函数在该点的变化率。
导数的计算方法有导数的定义、导数的几何意义和导数的物理意义。
3. 微分微分是导数的另一种表现形式,表示函数在某一点的局部变化量。
微分的计算方法有微分的定义、微分的几何意义和微分的物理意义。
4. 不定积分不定积分是求原函数的过程,分为基本不定积分和复合不定积分。
不定积分的计算方法有换元法、分部积分法和有理函数积分法。
5. 定积分定积分是求曲线下面积的过程,分为不定积分和定积分。
定积分的计算方法有牛顿-莱布尼茨公式、数值积分法和几何应用。
二、AP微积分BC1. 多元函数微分学多元函数是指有两个或两个以上自变量的函数。
多元函数的极限、连续性、偏导数、全微分等概念与单变量函数类似,但需要考虑多个自变量之间的关系。
2. 多元函数积分学多元函数的积分是指求多元函数在某一区域内的平均值或总和。
多元函数的重积分、多重积分和曲线积分等概念与单变量函数类似,但需要考虑多个自变量之间的关系。
3. 微分方程微分方程是描述变量之间关系的方程,分为常微分方程和偏微分方程。
常微分方程的解法有分离变量法、一阶线性微分方程、二阶常系数齐次线性微分方程和二阶常系数非齐次线性微分方程等。
偏微分方程的解法有分离变量法、格林公式、高斯公式等。
AP考试摸底考试题-微积分AB- AP Calculus-AB

46.
13.
30.
47.
14.
31.
48.
15.
32.
49.
16.
33.
50.
17.
34.
20 STEP 2. Determine Your Test Readiness
3.1 Getting Started!
Taking the Diagnostic Test helps you assess your strengths and weaknesses as you begin preparing for the AP Calculus AB exam. The questions in the Diagnostic Test contain both multiple-choice and open-ended questions. They are arranged by topic and designed to review concepts tested on the AP Calculus AB exam. All questions in the diagnostic test should be done without the use of a graphing calculator, except in a few cases where you need to find the numerical value of a logarithmic or exponential function.
17. How many points of inflection does the graph of y = sin(x 2) have on the interval [−π, π ]?
2014AP微积分CALCULUS知识点总结
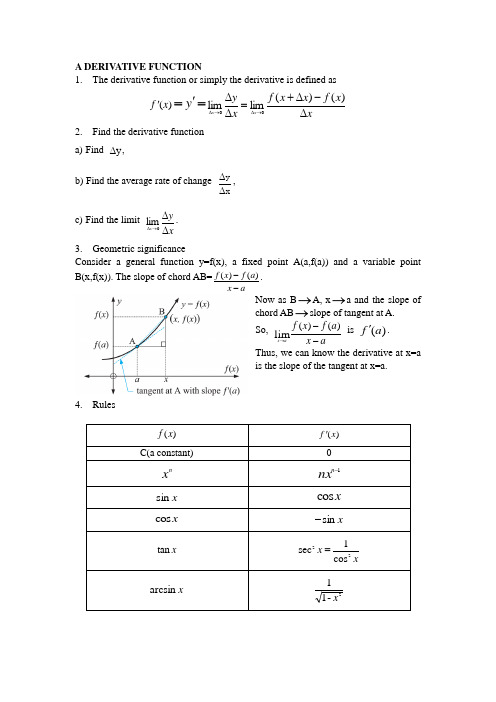
A DERIV ATIVE FUNCTION1. The derivative function or simply the derivative is defined as)(x f '=y '=xx f x x f x y x x ∆-∆+=∆∆→∆→∆)()(lim lim002. Find the derivative function a) Find y ∆,b) Find the average rate of change x y ∆∆, c) Find the limit xy x ∆∆→∆0lim .3. Geometric significanceConsider a general function y=f(x), a fixed point A(a,f(a)) and a variable point B(x,f(x)). The slope of chord AB=ax a f x f --)()(.Now as B →A, x →a and the slope of chord AB →slope of tangent at A. So, ax a f x f a x --→)()(lim is )(a f '.Thus, we can know the derivative at x=a is the slope of the tangent at x=a.4. Rules)(x f)(x f 'C(a constant) 0n x1-n nxx sinx cosx cosx sin -x tanxx 22cos 1sec =x arcsin2-11xx lnx 1x a loge xa log 1x e x e x aa a x ln )()(x v x u ±)()(x v x u '±')()(x v x u )()()()(x v x u x v x u '+')()(x v x u 2vv u v u '-' )0(≠v5. The chain ruleIf )(u f y = where )(x u u = thendxdu du dy dx dy =. )()(x g e x f = )()()(x g e x f x g '=' )(ln )(x g x f = )()()(x g x g x f '=' )(ln )()(ln )()()()(x u x v x u x v e e x u x f x v ===,])()()()(ln )([)()(ln )(x u x u x v x u x v ex f x u x v '+'='6. Inverse function, Parametric function and Implicit function Inverse function:dy dx dx dy 1=, ])([1)(1'='-x f x f , i.e., x y arcsin =, y x sin =Parametric function:dtdx dtdy dx dy =, i.e., )(t y ϕ=,)(t x ψ=→)(1x t -=ψ, )]([1x y -=ψϕ)()(t t dt dx dt dy dx dt dt dy dx dy ψϕ''=== Implicit function: 0))(,(=x y x F , 0))(,(=x f x F .0-222=+a y x ,ta y t a x sin cos ==, t ]2,0[π∈t ta t a dx dy x y cot sin cos )(-=-=='7. High derivativexx f x x f dx y d x f x ∆'-∆+'==''→∆)()(lim )(022 ta t a t dt dx dt y d dx y d x y x y x 32sin 1sin csc ])([)(-=-='='=''='' xx f x x f x f n n x n ∆-∆+=--→∆)()(lim )()1()1(0)( y=sinx )2sin(cos π+=='x x y , )22sin()2cos(ππ⨯+=+=''x x y )2sin()(π⨯+=n x ynB APPLICATIONS OF DIFFERENTIAL CALCULUS1. Monotonicitya) If S is an interval of real numbers and f(x) is defined for all x in S, then :f(x) is increasing on S ⇔ 0)(≥'x f for all x in S, and f(x) is decreasing on S ⇔0)(≤'x f for all x in S. b) Find the monotone interval ● Find domain of the function,● Find )(x f ', and x which make 0)(='x f , ● Draw sign diagram, find the monotone interval. 2. Maxima/Minima, Horizontal inflection, Stationary pointC INTEGRAL1. The idea of definite integralWe define the unique number between all lower and upper sums as⎰badx x f )( and call it “the definite integral of )(x f from a to b ”,i.e., ∑∑⎰=-=∆〈〈∆ni i n i ba i x x f dx x f x x f 110)()()( where nab x -=∆.We note that as ∞→n ,∑⎰-=→∆10)()(n i ba idx x f x x f and⎰∑→∆=ba ni i dx x f x x f )()(1We write ⎰∑=∆=∞→ba ni i n dx x f x x f )()(lim 1. If 0)(≥x f for all x on [a,b] then⎰badx x f )( is the shaded area.2. Properties of definite integrals⎰⎰-=-bab adx x f dx x f )()]([⎰⎰=ba b a dx x f c dx x cf )()(, c is any constant ⎰⎰⎰=+ca ba cb dx x f dx x f dx x f )()()( ⎰⎰⎰+=+bababadx x g dx x f dx x g x f )()()]()([)()()()(a F b F x F dx x f bab a-==⎰, where ⎰=dx x f x F )()(⎰-=a adx x f 0)((f(x) odd ),⎰⎰-=a aadx x f dx x f 0)(2)((f(x)even)If 0)(≥x f on b x a ≤≤ then⎰≥badx x f 0)(If )()(x g x f ≥ on b x a ≤≤ then⎰⎰≥b a ba dx x g dx x f )()(The average value of a function on an interval [a,b]⎰-=ba avedx x f ab f )(13. The infinite integral If )()(x f x F =', then ⎰+=C x F dx x f )()(Formulas:⎰++=+C x n dx x n n111, C a a dx a xx +=⎰ln 1 ⎰+-=C x inxdx cos s ,⎰+=Cx xdx sin cos ,C x xdx +-=⎰cos ln tan ,⎰+=C x xdx sin ln cotC x xdx +=-⎰arcsin 12(12<x ), C x x dx +=+⎰arctan 12 U Substitution⎰'dx x g x g f )())(( substitution u=g(x) ⎰du u f )(Integration by Parts⎰⎰-=vdu uv udv。
AP Calculus AB BC考点总结

AP微积分BC(包含AB)考点梳理嗨,少年/少女,无论你是自学的,还是在哪里学的,学完AP微积分AB或者BC,在你“婶婶”的脑海里应该有的知识框架是:函数的极限是什么概念,基本的计算方法和逻辑是怎样的;什么叫函数的连续,闭区间连续的函数有什么性质;导数是什么,有哪些常见的计算方法,有哪些基本应用;积分里的定积分和不定积分各自是什么,怎么计算,有什么应用;无穷级数是什么鬼,什么叫做无穷级数的收敛/发散,常见的无穷级数有哪些,常见判断无穷级数是否收敛的方法有哪些;幂级数是什么鬼,什么是它的收敛域(半径);泰勒级数/泰勒多项式是啥,用泰勒多项式进行估算时,误差边界怎么算。
具体要求内容如下:1.极限(Limits)1)极限定义的理解极限的逻辑,左右极限的概念以及此基础上的极限存在原则;还需要会从图像上判断极限。
2)基本计算一些基本函数的极限结论要熟悉,如y=e!在x分别趋向于正无穷、负无穷时的极限,y=sin x在x趋向于无穷大时的极限,等等;基本的加减乘除原则;有理函数类型(自变量趋向于无穷时,直接看最高项次方的关系,包括 e 3x!e!2x2e!e这种类似形式的);两个极限小公式(一个是sin x/x,一个是结果记为e的那个);洛比达法则(L’ Hopital’s Rule)——AB暂时不考——BC考极限喜欢考它。
3)求函数渐近线水平的和竖直的各自用极限是怎么定义计算的,基础还是极限计算。
不要死背公式,回到逻辑上去看。
2.连续(Continuity)1)连续的定义包括在一点的连续和在一个区间的连续的定义,以及如何根据定义去判断函数在一点是否连续(包括代数计算和根据图像的判断)。
2)闭区间连续函数的性质定理最值定理(Extreme Value Theorem)介值定理(Intermediate Value Theorem)零点定理(Zero Point Theorem)记住这三个定理的内容,理解其逻辑,并会联系Mean Value Theorem。
AP Calculus中的积分方法总结

AP Calculus中的积分方法总结AP频道为大家带来AP Calculus中的积分方法总结一文,希望对大家AP备考有所帮助。
1. 常见公式首先第一波是希望大家一定要牢记的公式每个都必须背起来!第二波公式属于:背不下来,你可以考场上临时推导一下嘛!下一篇推送我们在讲到具体方法的时候在三角函数那一块会来和大家讨论这些式子如何推导。
知道推导方法了以后,我们也可以考场上临时求一下。
2. 换元法一般常见的换元法,就不多说了,看到式子不熟悉的情况下,可以尝试用换元来做,但是换元如何选择,选择的好不好也影响到了这道题能不能做出来,方法是否简单。
比如下面这个式子:如何选择换元呢?你有以下几种选择:怎么选择才是最方便的呢?如何选择换元呢?总不能考试的时候慢慢试探吧。
所以希望大家能够熟练的掌握下一种方法:凑微分法!3. 凑微分法什么时候使用凑微分的方法?就是当你看到积分式子中有这样的形式可以去凑,并且剩余的部分只和右边括号里面的式子有关系,那么就可以用这样凑微分的方法来计算。
比如回到我们刚才的式子:如果稍微做出一些变形后,大家可以看到式子可以被变换成:可以把一个对x积分的式子变成对tanx积分的式子,同时我们可以观察到,剩下来的部分都是和tanx有关的部分,因此就可以把tanx看成是一个整体来处理。
这里如果用换元法去做的话,其实是我们把tanx看成了一个整体进行换元。
那么怎么知道这才是正确的换元方法呢?你得对上面的十个式子非常熟悉才可以吧!4. 一些特殊形式的规律1.多项式分式如果分母相对来说比较简单(什么叫分母简单呢,就是你把分子全部换成1以后,这样的分式你会积分计算,那就可以判断成分母较为简单)如这样的一些分母:这些分母形式都是可以直接套用公式,或者通过简单的换元/凑系数的方法进行快速的积分,因此我们把他们归成简单的分母。
(1)如果分子的最高次数大于等于分母的最高次数the highest order of the numerator is greater than or equal to the highest order of the denominator比如这样的:分子的最高次数都要大于等于分母的最高次数:我们采取的方法是:拆分子也就是把分子拆成多项来和分母约分,从而让最后的分式只保留分子较为简单的形式:(2)如果分母相对来说比较简单,但是分子的次数较小这个时候我们需要对分母进行处理,如果分母出现是二次多项式的形式我们可以把分母根据不同形式分成两种类型如果分母是第一种形式,我们把积分式子往arctan(x)的公式上去凑,比如:如果分母是第二种形式,我们需要进行因式分解,比如:不管分子是简单的1,还是关于x的简单的低次多项式,都可以采取这个方法。
ap微积分ab学

ap微积分ab学微积分(Calculus)是研究变化和速率的数学学科。
它涉及到两个主要的分支,分别是微分学和积分学。
微分学关注函数的变化率,而积分学关注函数的累积效应。
本文将介绍微积分的基本概念、原理和应用。
一、微积分的基本概念1. 函数(Function):函数是一种输入和输出之间的关系。
在微积分中,常用的函数包括多项式函数、三角函数和指数函数等。
2. 导数(Derivative):导数描述了函数在某一点的变化率。
它可以用来求解函数的极值点、判断函数的增减性等。
导数的计算方法包括使用极限的定义、利用导数的基本性质和使用导数的运算法则等。
3. 微分(Differential):微分是导数的一种应用形式,它描述了函数在某一点附近的线性近似。
4. 积分(Integral):积分是函数的反导数。
它可以求得曲线与坐标轴所围成的面积,也可以用来计算函数的累积效应。
积分的计算方法包括定积分和不定积分两种。
二、微积分的原理和公式1. 极限(Limit):极限是微积分的基本概念之一,用于描述函数在某一点的变化趋势。
常见的极限公式包括四则运算的性质、函数的性质和级数的性质等。
2. 重要的导数公式:(1) 常数函数的导数为零;(2) 幂函数的导数:$(x^n)' = nx^{n-1}$;(3) 指数函数的导数:$(a^x)' = a^x\ln(a)$;(4) 对数函数的导数:$(\log_a{x})' = \frac{1}{x\ln(a)}$;(5) 三角函数的导数公式;(6) 反函数的导数:若$y=f^{-1}(x)$,则$(f^{-1}(x))' =\frac{1}{f'(f^{-1}(x))}$。
3. 重要的积分公式:(1) 幂函数的积分:$\int{x^n}dx = \frac{1}{n+1}x^{n+1}+C$;(2) 指数函数和对数函数的积分公式;(3) 三角函数的积分公式;(4) 分部积分法:$\int{u \cdot v}dx = uv - \int{v \cdot du}$;(5) 曲线下面积的计算:$\int_{a}^{b}f(x)dx$。
最新-AP微积分CALCULUS知识点总结
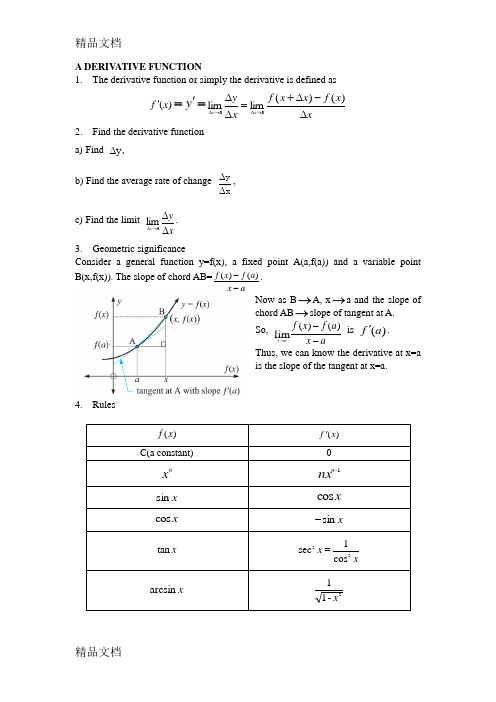
A DERIV ATIVE FUNCTION1. The derivative function or simply the derivative is defined as)(x f '=y '=xx f x x f x y x x ∆-∆+=∆∆→∆→∆)()(lim lim002. Find the derivative function a) Find y ∆,b) Find the average rate of change x y ∆∆, c) Find the limit xy x ∆∆→∆0lim .3. Geometric significanceConsider a general function y=f(x), a fixed point A(a,f(a)) and a variable point B(x,f(x)). The slope of chord AB=ax a f x f --)()(.Now as B →A, x →a and the slope of chord AB →slope of tangent at A. So, ax a f x f a x --→)()(lim is )(a f '.Thus, we can know the derivative at x=a is the slope of the tangent at x=a.4. Rules)(x f)(x f 'C(a constant) 0n x1-n nxx sinx cosx cosx sin -x tanxx 22cos 1sec =x arcsin2-11x5. The chain ruleIf )(u f y = where )(x u u = thendxdu du dy dx dy =. )()(x g e x f = )()()(x g e x f x g '=' )(ln )(x g x f = )()()(x g x g x f '=' )(ln )()(ln )()()()(x u x v x u x v e e x u x f x v ===,])()()()(ln )([)()(ln )(x u x u x v x u x v ex f x u x v '+'='6. Inverse function, Parametric function and Implicit function Inverse function:dy dx dx dy 1=, ])([1)(1'='-x f x f , i.e., x y arcsin =, y x sin =Parametric function:dtdx dtdy dx dy =, i.e., )(t y ϕ=,)(t x ψ=→)(1x t -=ψ, )]([1x y -=ψϕ)()(t t dt dx dt dy dx dt dt dy dx dy ψϕ''=== Implicit function: 0))(,(=x y x F , 0))(,(=x f x F .0-222=+a y x ,ta y t a x sin cos ==, t ]2,0[π∈t ta t a dx dy x y cot sin cos )(-=-=='7. High derivativexx f x x f dx y d x f x ∆'-∆+'==''→∆)()(lim )(022 ta t a t dt dx dt y d dx y d x y x y x 32sin 1sin csc ])([)(-=-='='=''='' xx f x x f x f n n x n ∆-∆+=--→∆)()(lim )()1()1(0)( y=sinx )2sin(cos π+=='x x y , )22sin()2cos(ππ⨯+=+=''x x y )2sin()(π⨯+=n x ynB APPLICATIONS OF DIFFERENTIAL CALCULUS1. Monotonicitya) If S is an interval of real numbers and f(x) is defined for all x in S,then :f(x) is increasing on S ⇔ 0)(≥'x f for all x in S, and f(x) is decreasing on S ⇔0)(≤'x f for all x in S. b)c) Find the monotone interval ●● Find domain of the function, ●● Find )(x f ', and x which make 0)(='x f , ● Draw sign diagram, find the monotone interval. 2. Maxima/Minima, Horizontal inflection, Stationary pointC INTEGRAL1. The idea of definite integralWe define the unique number between all lower and upper sums as⎰badx x f )( and call it “the definite integral of )(x f from a to b ”,i.e., ∑∑⎰=-=∆〈〈∆ni i n i ba i x x f dx x f x x f 11)()()( where nab x -=∆.We note that as ∞→n , ∑⎰-=→∆10)()(n i ba i dx x f x x f and⎰∑→∆=bani idx x f x x f )()(1We write ⎰∑=∆=∞→ba ni i n dx x f x x f )()(lim 1.If 0)(≥x f for all x on [a,b] then⎰badx x f )( is the shaded area.2. Properties of definite integrals⎰⎰-=-ba b a dx x f dx x f )()]([⎰⎰=babadx x f c dx x cf )()(, c is any constant⎰⎰⎰=+cab ac bdx x f dx x f dx x f )()()(⎰⎰⎰+=+ba b a b a dx x g dx x f dx x g x f )()()]()([)()()()(a F b F x F dx x f baba-==⎰, where ⎰=dx x f x F )()(⎰-=a adx x f 0)((f(x) odd ),⎰⎰-=a aadx x f dx x f 0)(2)((f(x)even)If 0)(≥x f on b x a ≤≤ then⎰≥badx x f 0)(If )()(x g x f ≥ on b x a ≤≤ then⎰⎰≥b abadx x g dx x f )()(The average value of a function on an interval [a,b]⎰-=ba avedx x f ab f )(13. The infinite integralIf )()(x f x F =', then⎰+=C x F dx x f )()(Formulas:⎰++=+C x n dx x n n111, C a a dx a x x+=⎰ln 1 ⎰+-=Cx inxdx cos s ,⎰+=Cx xdx sin cos ,C x xdx +-=⎰cos ln tan ,⎰+=C x xdx sin ln cotC x xdx +=-⎰arcsin 12(12<x ), C x x dx +=+⎰arctan 12 U Substitution⎰'dx x g x g f )())(( substitution u=g(x) ⎰du u f )(Integration by Parts⎰⎰-=vdu uv udv。
AP Calculus AB review AP微积分复习提纲PDF.pdf

AP CALCULUS AB REVIEWChapter 2DifferentiationDefinition of Tangent Line with Slop mIf f is defined on an open interval containing c, and if the limitexists, then the line passing through (c, f(c)) with slope m is the tangentline to the graph of f at the point (c, f(c)).Definition of the Derivative of a FunctionThe Derivative of f at x is given byprovided the limit exists. For all x for which this limit exists, f’is afunction of x.*The Power Rule*The Product Rule***The Chain Rule☺Implicit Differentiation (take the derivative on both sides; derivativeof y is y*y’)Chapter 3Applications of Differentiation*Extrema and the first derivative test (minimum: − → + , maximum: +→ −, + & − are the sign of f’(x) )*Definition of a Critical NumberLet f be defined at c. If f’(c) = 0 OR IF F IS NOT DIFFERENTIABLEAT C, then c is a critical number of f.*Rolle’s TheoremIf f is differentiable on the open interval (a, b) and f (a) = f (b), then thereis at least one number c in (a, b) such that f’(c) = 0.*The Mean Value TheoremIf f is continuous on the closed interval [a, b] and differentiable on theopen interval (a, b), then there exists a number c in (a, b) such that f’(c) = .*Increasing and decreasing interval of functions (take the first derivative)*Concavity (on the interval which f’’ > 0, concave up)*Second Derivative TestLet f be a function such that f’(c) = 0 and the second derivative of f existson an open interval containing c.1.If f’’(c) > 0, then f(c) is a minimum2.If f’’(c) < 0, then f(c) is a maximum*Points of Inflection (take second derivative and set it equal to 0, solve theequation to get x and plug x value in original function)*Asymptotes (horizontal and vertical)*Limits at Infinity*Curve Sketching (take first and second derivative, make sure all thecharacteristics of a function are clear)♫ Optimization Problems*Newton’s Method (used to approximate the zeros of a function, which istedious and stupid, DO NOT HA VE TO KNOW IF U DO NOT WANTTO SCORE 5)Chapter 4 & 5Integration*Be able to solve a differential equation*Basic Integration Rules1)2)3)4)*Integral of a function is the area under the curve*Riemann Sum (divide interval into a lot of sub-intervals, calculate the area for each sub-interval and summation is the integral).*Definite integral*The Fundamental Theorem of CalculusIf a function f is continuous on the closed interval [a, b] and F is an anti-derivative of f on the interval [a, b], then.*Definition of the Average Value of a Function on an IntervalIf f is integrable on the closed interval [a, b], then the average value off on the interval is.*The second fundamental theorem of calculusIf f is continuous on an open internal I containing a, then, for every x in the interval,.*Integration by Substitution*Integration of Even and Odd Functions1) If f is an even function, then.2) If f is an odd function, then.*The Trapezoidal RuleLet f be continuous on [a, b]. The trapezoidal Rule for approximating is given byMoreover, a n →∞, the right-hand side approaches.*Simpson’s Rule (n is even)Let f be continuous on [a, b]. Simpson’s Rule for approximating isMoreover, as n→∞, the right-hand side approaches*Inverse functions(y=f(x), switch y and x, solve for x)*The Derivative of an Inverse FunctionLet f be a function that is differentiable on an interval I. If f has an inverse function g, then g is differentiable at any x for which f’(g(x))≠0. Moreover,, f’(g(x))≠0.*The Derivative of the Natural Exponential FunctionLet u be a differentiable function of x.1. 2..*Integration Rules for Exponential FunctionsLet u be a differentiable function of x..♠Derivatives for Bases other than eLet a be a positive real number (a ≠1) and let u be a differentiable function of x.1. 2.♠♠*Derivatives of Inverse Trigonometric FunctionsLet u be a differentiable function of x.*Definition of the Hyperbolic Functions。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
AP CALCULUS AB REVIEWChapter 2DifferentiationDefinition of Tangent Line with Slop mIf f is defined on an open interval containing c, and if the limitlim ∆x→0∆y∆x=lim∆x→0f(c+∆x)−f(c)∆x=mexists, then the line passing through (c, f(c)) with slope m is the tangent line to the graph of f at the point (c, f(c)).Definition of the Derivative of a FunctionThe Derivative of f at x is given byf′(x)=lim∆x→0f(c+∆x)−f(c)∆xprovided the limit exists. For all x for which this limit exists, f’is afunction of x.*The Power Rule*The Product Rule*ddx[sin x]=cos x*ddx[cos x]=−sin x*The Chain Rule☺Implicit Differentiation (take the derivative on both sides; derivativeof y is y*y’)Chapter 3Applications of Differentiation*Extrema and the first derivative test (minimum: − → + , maximum: +→ −, + & − are the sign of f’(x) )*Definition of a Critical NumberLet f be defined at c. If f’(c) = 0 OR IF F IS NOT DIFFERENTIABLEAT C, then c is a critical number of f.*Rolle’s TheoremIf f is differentiable on the open interval (a, b) and f (a) = f (b), then thereis at least one number c in (a, b) such that f’(c) = 0.*The Mean Value TheoremIf f is continuous on the closed interval [a, b] and differentiable on theopen interval (a, b), then there exists a number c in (a, b) such that f’(c) =f(b)− f(a).b−a*Increasing and decreasing interval of functions (take the first derivative)*Concavity (on the interval which f’’ > 0, concave up)*Second Derivative TestLet f be a function such that f’(c) = 0 and the second derivative of f existson an open interval containing c.1.If f’’(c) > 0, then f(c) is a minimum2.If f’’(c) < 0, then f(c) is a maximum*Points of Inflection (take second derivative and set it equal to 0, solve theequation to get x and plug x value in original function)*Asymptotes (horizontal and vertical)*Limits at Infinity*Curve Sketching (take first and second derivative, make sure all thecharacteristics of a function are clear)♫ Optimization Problems*Newton’s Method (used to approximate the zeros of a function, which istedious and stupid, DO NOT HA VE TO KNOW IF U DO NOT WANTTO SCORE 5)Chapter 4 & 5Integration*Be able to solve a differential equation*Basic Integration Rules1)∫u ndu =u n+1n+1+ C,n ≠−12)∫sin u du = −cos u + C 3)∫cos u du = sin u + C 4)∫1u du = ln u*Integral of a function is the area under the curve*Riemann Sum (divide interval into a lot of sub-intervals, calculate thearea for each sub-interval and summation is the integral).*Definite integral*The Fundamental Theorem of CalculusIf a function f is continuous on the closed interval [a, b] and F is ananti-derivative of f on the interval [a, b], then ∫f (x )dx ba=F (b )− F(a).*Definition of the Average Value of a Function on an IntervalIf f is integrable on the closed interval [a, b], then the average value off on the interval is 1b−a ∫f(x)dx ba.*The second fundamental theorem of calculusIf f is continuous on an open internal I containing a, then, for every x in the interval,ddx[∫f (t )dt x a ]=f(x).*Integration by Substitution*Integration of Even and Odd Functions1) If f is an even function, then ∫f (x )dx b a =2∫f(x)dx ba . 2) If f is an odd function, then ∫f (x )dx ba=0.*The Trapezoidal RuleLet f be continuous on [a, b]. The trapezoidal Rule forapproximating ∫f (x )dx bais given by ∫f (x )dx ba≈ b−a 2n[f (x 0)+2f (x 1)+2f (x 2)+⋯+2f (x n−1) +f (x n )]Moreover, a n → ∞, the right-hand side approaches ∫f (x )dx ba. *Simpson ’s Rule (n is even)Let f be continuous on [a, b]. Simpson ’s Rule for approximating∫f (x )dx bais∫f (x )dx ba≈b −a3n [f (x 0)+4f (x 1)+2f (x 2)+4f (x 3)+⋯4f (x n−1)+f (x n )]Moreover, as n →∞, the right-hand side approaches∫f (x )dx ba*Inverse functions(y=f (x), switch y and x, solve for x)*The Derivative of an Inverse FunctionLet f be a function that is differentiable on an interval I . If f has aninverse function g , then g is differentiable at any x for which f ’(g (x))≠0. Moreover, g ′(x )=1f ′(g(x)), f ’(g (x))≠0.*The Derivative of the Natural Exponential Function Let u be a differentiable function of x .1.d dx[e x ]= e x 2.d dx[e u ]= e udu dx.*Integration Rules for Exponential Functions Let u be a differentiable function of x. ∫e u du = e u +C .♠Derivatives for Bases other than eLet a be a positive real number (a ≠1) and let u be a differentiablefunction of x . 1.d dx[a u ]=(ln a)a udu dx2.ddx[log a u ]=1u ln a dudx♠∫a x dx =(1ln a)a x +C ♠lim x→∞(1+1x)x =lim x→∞(x+1x)x=e*Derivatives of Inverse Trigonometric Functions Let u be a differentiable function of x.d dx [sin−1u]=√2ddx[cos−1u]=√2d dx [tan−1u]=u′1+u2√22=sin−1ua+C∫dua2+u2=1atan−1ua+C√22=1asec−1|u|a+C*Definition of the Hyperbolic Functionssinh x=e x−e−x2cosh x=ex+e−x2tanh x=sinh xcosh x csch x=1sinh x,x≠0sech x=1cosh x coth x=1tanh x,x≠0。
