大学数学专业英语第一章

合集下载

数学专业英语第三版课文翻译章

数学专业英语第三版课文翻译章本文将根据数学专业英语第三版课文《Step by Step Thinking》进行翻译。

"Step by Step Thinking"is an article that introduces the concept of step-by-step thinking in mathematics.It highlights the importance of breaking down complex problems into smaller,more manageable steps in order to solve them effectively.The article begins by stating that step-by-step thinking is a fundamental skill in mathematics.It emphasizes the need to approach problems by breaking them downinto smaller components,as this helps to clarify the problem and identify potential solutions.The author argues that this approach is not only applicable tomathematics but also to various other fields,as it promotes clearer thinking and problem-solving abilities.The article then discusses the step-by-step thinking process in more detail.It suggests that the first step is tocarefully read and understand the problem, ensuring that all relevant information is identified.This is followed by breaking the problem down into smaller sub-problems or steps,each of which can be solved individually.The author emphasizes the need to be systematic and organized during this process,as it helps to prevent mistakes and confusion.Furthermore,the article highlights the importance of logical reasoning in step-by-step thinking.It states that each step should be justified with logical reasoning,ensuring that the solution is based on sound mathematical principles.The author advises against skipping steps or making assumptions without proper justification,as this can lead to erroneous results.The article also provides examples to illustrate the step-by-step thinking approach.It presents a complex problem and demonstrates how breaking it down into smaller steps can simplify the solution process.By solving each step individually and logically connecting them,the problem can be solved effectively.In conclusion,"Step by Step Thinking" emphasizes the significance of step-by-step thinking in mathematics and problem-solving. It encourages readers to approach problems systematically,breaking them down into smaller components,and justifying eachstep with logical reasoning.This approach promotes clearer thinking and enhances problem-solving abilities,not only in mathematics but also in other disciplines.。

高数第一章知识点总结

高数第一章知识点总结希望同学们在准备考研数学高数的复习过程中能够适当结合真题与模拟题，下面是精心收集的高数第一章知识点总结，希望能对你有所帮助。

篇一：高数第一章知识点总结高等数学是考研数学的重中之重，所占的比重较大，在数学一、三中占56%，数学二中占78%，重点难点较多。

具体说来，大家需要重点掌握的知识点有几以下几点：1.函数、极限与连续：主要考查极限的计算或已知极限确定原式中的常数;讨论函数连续性和判断间断点类型;无穷小阶的比较;讨论连续函数在给定区间上零点的个数或确定方程在给定区间上有无实根。

2.一元函数微分学：主要考查导数与微分的定义;各种函数导数与微分的计算;利用洛比达法则求不定式极限;函数极值;方程的的个数;证明函数不等式;与中值定理相关的证明;最大值、最小值在物理、经济等方面实际应用;用导数研究函数性态和描绘函数图形;求曲线渐近线。

3.一元函数积分学：主要考查不定积分、定积分及广义积分的计算;变上限积分的求导、极限等;积分中值定理和积分性质的证明;定积分的应用，如计算旋转面面积、旋转体体积、变力作功等。

4.多元函数微分学：主要考查偏导数存在、可微、连续的判断;多元函数和隐函数的一阶、二阶偏导数;多元函数极值或条件极值在与经济上的应用;二元连续函数在有界平面区域上的最大值和最小值。

此外，数学一还要求会计算方向导数、梯度、曲线的切线与法平面、曲面的切平面与法线。

5.多元函数的积分学：包括二重积分在各种坐标下的计算，累次积分交换次序。

数一还要求掌握三重积分，曲线积分和曲面积分以及相关的重要公式。

6.微分方程及差分方程：主要考查一阶微分方程的通解或特解;二阶线性常系数齐次和非齐次方程的特解或通解;微分方程的建立与求解。

差分方程的基本概念与一介常系数线形方程求解方法由于微积分的知识是一个完整的体系，考试的题目往往带有很强的综合性，跨章节的题目很多，需要考生对整个学科有一个完整而系统的把握。

最后凯程考研名师预祝大家都能取得好成绩。

数学专业英语第一讲基础知识与基本特点

Multiply three by five , you get fifteen . Three times five is (makes, will be , equals, is equal to ) fifteen .
13
English for Mathematics
4．除：用divide·by·/ divided by / · · · · divide·into· / into 等表示。 · · · ·
10 English for Mathematics
基本数学符号的表示法：
1．加：用and ,plus , added to 等词表示。
2 ＋ 4 ＝ 6 可译为：
Two and four is six . Two plus four is equal to six . Two added to four equals six . Two and four makes six . Two plus four will be six . If you add two to four , you get six .
3
English for Mathematics
由于数学内容的英语表达有其特殊性，阅读时不仅要靠个人在公共英语上的基本功，而且还要靠数学基本知识的掌握及其运用的能力。
4
English for Mathematics
开设本课程目的
1、为数学专业研究生阅读数学英文文章和发表数学英文文章打基础，同时扩大学生英语知识面, 为相关专业的研究生做英语保温和一定的强化。
English for Mathematics
常用标点符号：
× ÷ ＝ ≠ is multiplied by 乘号 is divided by 除号 is equal to 等于号 is not equal to 不等于号

高一英语大学专业选择指导练习题40题

高一英语大学专业选择指导练习题40题1. Which major is related to the study of living organisms?A. Computer ScienceB. BiologyC. MathematicsD. History答案解析：B。

Biology 是生物学，主要研究生物，与living organisms（生物体）相关。

Computer Science 是计算机科学，与生物体无关。

Mathematics 是数学，也不涉及生物体研究。

History 是历史，同样与生物体没有关系。

2. Which major focuses on the design and development of software?A. PhysicsB. ChemistryC. EngineeringD. Computer Science答案解析：D。

Computer Science 是计算机科学，专注于软件的设计和开发。

Physics 是物理学，与软件设计无关。

Chemistry 是化学，也不涉及软件。

Engineering 是工程学，范围比较广，但不是专门针对软件设计和开发。

3. Which major is concerned with the study of human behavior and mental processes?A. PsychologyB. EconomicsC. SociologyD. Literature答案解析：A。

Psychology 是心理学，研究人类行为和心理过程。

Economics 是经济学，主要研究经济现象。

Sociology 是社会学，关注社会现象。

Literature 是文学，与人类行为和心理过程的研究关系不大。

4. Which major deals with the management of financial resources?A. AccountingB. MarketingC. PhilosophyD. Art答案解析：A。

大学化学专业英语PPT课件第一章 Chapter1

李阳经典理论精选：
Don't be shy, just try. I enjoy losing face. I enjoy being embarrassed. I enjoy being laughed at. I enjoy being made fun of. I enjoy making mistakes. I enjoy learning from my mistakes! The more mistakes I make, the more progress I make! Try to lose face as much as possible! Try to make people laugh as much as possible! Try to be embarrassed as much as possible! Try to make progress as much as possible! Start to make mistakes and lose face today! That's the only way to success!
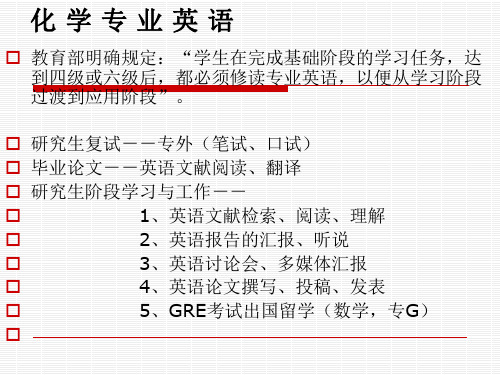
化学专业英语的特点
1、英语（表达、思维、语言文字）单词、语法、课文、习题 2、专业性：词汇：化学专业词汇课文：（1）化学相关的文章（2）英文原版教材（3）化学计算的英文表述
教学方式：

1、中英文双语讲授 2、多媒体教学专业词汇：英文解释专业文献：学生朗读、难点解释习题：英文提问、英文回答讨论交流：化学专业英语角(CSEC) Chemistry Speciality English Corner 化学专业英语竞赛：科技部主办 Chemistry Speciality English Society

关于数学专业英语课程教学的一些探讨与体会

关于数学专业英语课程教学的一些探讨与体会【摘要】数学专业英语课程是面向数学系学生所开始的一门专业任选课，它具有英语、数学、英语与数学相结合等特点，要求学生具备一定的数学知识和英语知识。

本文针对数学专业英语教学的现状，结合此门课程所具有的特点，来探讨如何提高学生学习数学专业英语的乐趣，提高学生阅读数学专业英语文献的能力，同时介绍一些数学的前沿研究热点。

【关键词】数学专业英语；教学探讨；教学方法如今越来越多的本科生毕业后到国外进行研究生学习，首要面对的一个紧要问题就是要听懂国外教师上课。

虽然在出国之前经历过各种各样的英语考试，口语和听力有了一定的提高，但专业英语词汇量比较缺乏。

数学专业英语课是针对数学系本科生所开设的一门专业任选课，课程开设的目的是帮助本科生能掌握一定的数学专业词汇，提高阅读数学专业文献的能力，促进我国的数学教育与国际上的交流，紧跟国际数学的研究步伐。

此课程的开设有助于扩大本科生的视野，知道一些数学专业词汇的英文表达，进一步会撰写一篇英语科技论文。

我们学院通常在第四学期开设专业英语选修课，从前两年的教学效果来看，绝大部分学生对此门课程兴趣不大，抱着拿两学分的心态，因此在课堂不能和教师进行良好的互动，课堂浑然无趣。

本文从以下四个方面指出在教学中所存在的一些问题，并探讨数学专业英语课程的教学改革方法与策略。

一、教学课时量较少目前学院采用吴炯圻老师的数学英语课本-第二版，此门课程性质是专业任选课，安排总课时为32。

课本内容总共6章，分别介绍了数学专业的阅读和翻译初阶；精读课文；专业文选；数学英语专业论文写作基础；查阅英语数学文献的基本知识和数学文献常用英语词汇。

从课本内容来看，涉及内容较多，如第二章内容有12小节，从课时安排来看，要想讲完全部内容有难度，因此在课堂教学中，我们选取了与数学分析、高等代数和概率论与数理统计等学科相关的内容来讲解，如函数的思想；线性空间中的相关与无关集；概率论与数理统计中的基本术语，通过讲解这些内容，让同学们能够联想到之前学过的数学内容，从而会主动用专业英语来表述。

大学专业常见课程英文词汇[最新]

大学专业常见课程英文词汇大学英语,,,,,,College English高等数学,,,,,,Advanced Mathematics体育,,,,,,,,,Physical Education军事理论,,,,,,Military Theory机械制图,,,,,,Mechanical Graphing算法语言,,,,,,Algorithmic Language大学物理,,,,,,College Physics物理实验,,,,,,Experiment of College Physics线性代数,,,,,,Linear Algebra法律基础,,,,,,Fundamentals of Law普通物理,,,,,,General Physics普通物理实验室,,,,,,Lab of General Physics复变函数与积分变换,,,Functions of Complex V ariables & Integral Transformati ons电路理论,,,,,,,,,Theory of Circuitry电路测试技术,,,,,,Circuit Measurement Technology概率论与随机过程,,,Probability Theory & Stochastic Process信号与线性系统,,,,,,Signal & Linear System电子线路,,,,,,,,,Circuitry脉冲与数字电路,,,,,,Pulse & Numerical Circuitry金工实习,,,,,,Metalworking Practice电工实习,,,,,,Electrical Engineering PracticeCET-4,,,College English Test (Band 4)电子线路实验,,,Experiment in Electronic Circuitry微机原理,,,,,,Principle of Microcomputer电磁场与电磁波Electromagnetic Fields & Magnetic Waves电机电器与供电Motor Elements and Power Supply计算方法,,,,,,Computational Method软件技术基础,,,Basis of Software Technique微波技术,,,,,,Microwave Technique通讯原理,,,,,,Principle of Communication数字信号处理,,,Digital Signal Processing微机实验,,,,,,Experiment of Microcomputer计算机接口技术Computer Interface Technologyc 语言,,,,,, C languageCET-6,,,College English Test (Band 6)工业企业管理,,,Industrial Enterprise Management移动通讯,,,,,,Moving Communication光纤通讯系统,,,Fiber Optical Communication System可靠性技术导论Introduction to Reliability Technology卫星通信,,,,,,Satellite Communications电视原理,,,,,,Television Operation数字图象处理,,,Digital Image Processing专业英语,,,,,,Specialty English情报检索,,,,,,Information Searches毕业设计,,,,,,Graduation Thesis自动控制理论,,,Automatic Control Theory模拟电子电路,,,Analogical Electronics数字电子电路,,,Digital Electronics资本主义经济,,,Economy of Capitalism马克思主义原理Principle of Marxism机械原理,,,,,,Principle of Mechanic机械设计,,,,,,Mechanic Design最优控制,,,,,,Optimum Control微机控制技术,,,Microcomputer Control Technology过程控制,,,,,,Procedure Control自动控制系统,,,Automatic Control System半导体变流技术Semiconductor converting Technique 运筹学,,,,,,Operational Research自动检测技术,,,Auto-Measurement Technique传感器原理,,,Principle of Sensing Device单片机原理,,,Principle of Single-Chip computer学科分类词汇Chinese语文English英语Japanese日语mathematics数学science理科gymnastics体育history历史algebra代数geometry几何geography地理biology生物chemistry化学biochemistry生物化学physics物理physical geography地球物理literature文学sociology社会学linguistics语言学psycology心理学philosophy哲学engineering工程学mechanical engineering机械工程学electronic engineering电子工程学medicine医学social science社会科学agriculture农学astronomy天文学economics经济学politics政治学comercial science商学biochemistry生物化学anthropology人类学languistics语言学accounting会计学law, jurisprdence法学banking银行学metallurgy冶金学finance财政学mass-communication大众传播学journalism新闻学atomic energy原子能学civil engineering土木工程architecture建筑学chemical, engineering化学工程accounting and satisics会计统计business administration工商管理library图书馆学diplomacy外交foreign language外文botany植物major主修。

数学与英语

数学是世界上最普遍、最实用的工具，也是一种语言，它的领域不仅仅局限于交流与沟通，是科学发展的标志，是大自然的破译密码，也是研究微观世界的重要工具。

数学在人类历史发展和社会生活中发挥着不可替代的作用，也是学习和研究现代科学技术必不可少的基本工具。

基础数学的知识与运用是个人与团体生活中不可或缺的一部分。

就像康托尔说过那样，数学的本质在于它的自由。

数学的自由体现在方方面面数学被应用在很多不同的领域上。

基础数学在生活中的运用范围广，与很多事物都有联系甚至是与其他学科有着密不可分的联系。

英语是当今世界上主要的国际通用语言之一，也是世界上最被广泛使用的第二语言，是欧盟和许多国际组织与英联邦国家的官方语言之一，也是联合国的工作语言之一。

英语的母语使用者数量位居世界第三，少于标准汉语和西班牙语，但上两个世纪英国和美国在文化、经济、军事、政治和科学上的领先地位使得英语成为一种国际语言，如今许多国际场合都使用英语作为沟通的媒介。

英语也是与电脑联系最密切的语言，大多数编程语言都与英语有联系，而且随着网络使用，使英文使用更为普及。

数学与英语是我们学习中最为基本也是最重要的两个学科。

这两个学科之间有着什么微小的联系，就是本论文主要介绍之处。

数学与英语有着非常紧密的关系，。

我校作为理工科院校，很多院系专业的学习经常运用到数学可以说每天的生活都离不开数学。

1《大学英语教学大纲》规定: 大学非英语专业的英语教学是由两个阶段来完成的, 即: 基础英语阶段和专业英语阶段, 其中专业英语是基础英语的后续课程. 就数学专业英语而言, 它是一门由本专业教师授课的涉及数学专业领域的英语教学课程. 学生通过大学1、2学年基础英语的学习, 在听、说、读、写、译等方面已经打下了一定的基础, 但离掌握英语的实用技能并灵活应用还有相当的一段差距, 数学专业英语教学在此基础上进一步提供了学习、使用、实践英语的课堂环境, 是学生结合专业知识使用英语, 培养英语的实用技能的重要环节. 学生通过数学专业英语的学习来掌握数学文章的文体、词法、词汇、表达、翻译、写作等方面的知识, 提高听、说、读、写、译等方面的基本交际技能, 培养学生利用英语交流和获取信息的能力和习惯, 为以后数学专业课的双语教学和未来从事中小学数学双语教学打下坚实的英语基础。

创新大学英语1教案unit1

教案2015 ～2016 学年第一学期分院（部) 数学分院专业数学教育课程名称大学英语1课程代码授课班级2015级汉数学教育普专班授课教师廖雪莲职称助教教材创新大学英语综合教程高职高专版Book1新疆教育学院教务处制课程教案授课题目（或章节）Book 1 Unit 1Reception课型课时10课时教学目标1. Teach the students how to pick up a client at the airport.2. Train the students’ ability to plan a business reception and know some intercultural reception skills.3. Give the students a chance to exchange views on the texts to enable them to havea deeper understanding of the texts and to let the students be acquainted with some new words.4.Teach the students some practical reading skills and writing skills.教学重点1.Knowing how to pick up a client at a airport.2. Email writingeful words and expressions: reception activity track flight pick up entertain available greet plan invite hospitality spa land serve baggage dinner specialty recommend see off celebrate教学难点1. Planning a business reception2. Tenses3. Writing an invitation via email教学方法Interactive approach Situational teaching approach Teamwork methodTask-based teaching method教具PPTBlackboard11. The first two-class-hour session for Warm-up & Unit task (Text A)2. The second two-class-hour session for Section A Tips for Business Reception3. The third two-class session for Section B Reading4. The fourth two-class-hour session for Exercises and grammar5. The last two-class-hour session for Listening comprehension and email writingWarm-up and Unit task (Text A)Step 1 Lead-in: Business reception1. Warm-up questions: What is business reception?A business reception means the first step o impress your client. A good reception can help the following negotiation and the following deals. Reception includes meeting the clients at the airport, reserving hotel rooms for clients, planning recreational activities to entertain clients and hosting a dinner for clients, too.2. The Ss read warm-up 1 and match the names of different activities with their pictures.3. Invite 1-2 Ss to present their answers.4.Invite 3-4 Ss to express their ideas according to the sample.Step 2 Warm-up 21. Ss read Warm-up22.Ask Ss to do a pair work. Try the quiz and check the score for their partner.3.Invite a student to present his/her partner’s result.4.The teacher help to explain some difficult language points.5.Introduce more intercultural business reception skills.Step 3 Assignments1. preview text ASection A Tips for Business ReceptionStep 1 Warm-up questions1. How to track flights status?2. How to pick up your clients?Step2 Reading231. Jigsaw reading let the students share the tips for business reception.2. Rank the steps of picking a client up at the airport.Step 3 Language PointsThe Ss choose some useful expressions and sentences from the passage, and then the teacher demonstrates their usage. The following should be chosen and practiced. The Ss should make up at least two sentences using the chosen phrases in groups.1. reception [ri'sep ʃən] n . 接见；接待；迎接 ;招待会；欢迎会 ;接纳；接受；容纳;【无线电、电视】接收；接收能力; (饭店、旅馆等处的)接待处，招待处 e.g. The reception is over.招待会到此结束。

大学数学专业英语教材

大学数学专业英语教材IntroductionMathematics plays a crucial role in various fields and industries, and studying mathematics at the university level requires a solid foundation in both the subject itself and the English language. A well-designed mathematics textbook for university students in the field of mathematics can effectively integrate mathematical concepts with English language learning. In this article, we will explore the essential features and requirements of a comprehensive English textbook for mathematics students at the university level.Chapter 1: Fundamental ConceptsThe first chapter of the textbook should cover the fundamental concepts of mathematics, introducing students to the basic principles that underpin the subject. It should provide concise explanations and definitions, supplemented with examples and illustrations to aid comprehension. Additionally, this chapter should include exercises to reinforce learning and promote critical thinking.Chapter 2: AlgebraAlgebra is a cornerstone of mathematics, and this chapter should delve into its key theories and principles. It should cover topics such as equations, inequalities, functions, and matrices. The textbook should present clear explanations of concepts, accompanied by real-life applications to demonstrate the practical relevance of algebra.Chapter 3: CalculusCalculus is essential for advanced mathematics and the study of other disciplines such as physics and engineering. The textbook should guide students through both differential and integral calculus, ensuring a thorough understanding of concepts like limits, derivatives, and integrals. Practical examples and exercises should be incorporated to enhance students' problem-solving skills.Chapter 4: Probability and StatisticsIn this chapter, the textbook should introduce students to probability theory and statistical analysis. The content should cover topics such as probability distributions, hypothesis testing, and regression analysis. The inclusion of real-world data sets and case studies can foster students' ability to apply statistical methods effectively.Chapter 5: Discrete MathematicsDiscrete mathematics is vital in areas like computer science and cryptography. This chapter should explore concepts such as set theory, logic, graph theory, and combinatorics. The textbook should present clear explanations of these topics, accompanied by relevant examples and exercises to consolidate understanding.Chapter 6: Linear AlgebraLinear algebra is widely applicable in various fields, including computer science and physics. This chapter should cover vector spaces, linear transformations, and eigenvalues. Emphasis should be placed on theconnections between linear algebra and other mathematical disciplines, demonstrating its practical significance.Chapter 7: Number TheoryNumber theory explores the properties and relationships of numbers, and it forms the basis for cryptographic algorithms and computer security systems. This chapter should introduce students to prime numbers, modular arithmetic, and cryptographic algorithms. Examples and exercises should be given to develop students' problem-solving skills in the realm of number theory.Chapter 8: Numerical AnalysisNumerical analysis involves using algorithms to solve mathematical problems on computers. This chapter should cover topics such as interpolation, numerical integration, and numerical solutions of equations. The textbook should provide step-by-step guidance on implementing numerical algorithms, allowing students to develop practical coding skills.ConclusionA comprehensive English textbook for university-level mathematics students should provide a solid foundation in mathematical concepts while simultaneously enhancing students' English language proficiency. By incorporating clear explanations, practical examples, and engaging exercises, this textbook can foster a deep understanding of mathematics within an English language learning context. Such a resource will empower students to pursue further studies in mathematics and apply their skills in various professional domains.。

高等数学英文教材百度百科

高等数学英文教材百度百科Higher Mathematics English Textbook on Baidu BaikeIntroductionBaidu Baike is an online encyclopedia platform that provides a wide range of information on various subjects. In this article, we will explore the topic of Higher Mathematics English Textbook on Baidu Baike, focusing on its content, structure, and significance. This English textbook plays a crucial role in helping students understand and master advanced mathematical concepts.ContentThe Higher Mathematics English Textbook on Baidu Baike covers a comprehensive range of topics, including calculus, linear algebra, differential equations, and probability theory. Each chapter delves into specific mathematical concepts and offers detailed explanations, examples, and practice exercises. The content is designed to progressively build upon previous knowledge, ensuring a smooth transition from basic to more advanced mathematical concepts.StructureThe textbook follows a logical structure, starting with fundamental mathematical principles and gradually progressing towards more complex topics. Each chapter begins with an introduction that provides an overview of the subject matter. The main content includes definitions, formulas, theorems, and proofs, presented in a concise and understandable manner.Additionally, the textbook incorporates numerous diagrams, graphs, and illustrations to aid in visual comprehension.SignificanceThe Higher Mathematics English Textbook on Baidu Baike holds great significance for several reasons. Firstly, it serves as a valuable resource for students studying higher mathematics in English-speaking countries or non-native English speakers pursuing mathematical studies in English. The textbook provides a comprehensive understanding of mathematical concepts while improving English language skills.Secondly, the textbook's availability on Baidu Baike ensures easy access for students, researchers, and educators. Baidu Baike is a reliable online platform that allows users to access information conveniently, making the Higher Mathematics English Textbook widely accessible.Furthermore, the use of an English textbook contributes to the globalization of mathematics education. By providing educational material in English, Baidu Baike bridges the gap between different mathematical communities worldwide, promoting cross-cultural collaboration and knowledge exchange.ConclusionIn conclusion, the Higher Mathematics English Textbook on Baidu Baike is an invaluable resource for students and academics alike. Its comprehensive content, well-structured format, and global accessibility make it an essential tool for anyone seeking to explore and master advanced mathematical concepts in English. By fostering an inclusive andcollaborative mathematics education environment, Baidu Baike plays a crucial role in advancing mathematical knowledge worldwide.。

数学专业英语(Doc版).9

学专业英语－How to Organize a paper (For Beginers)?The usual journal article is aimed at experts and near-experts, who are the peo ple most likely to read it. Your purpose should be say quickly what you have done is good, and why it works. Avoid lengthy summaries of known results, and minimize the preliminaries to the statements of your main results. There ar e many good ways of organizing a paper which can be learned by studying pa pers of the better expositors. The following suggestions describe a standard acc eptable style.Choose a title which helps the reader place in the body of mathematics. A use less title: Concerning some applications of a theorem of J. Doe. A. good title contains several well-known key words, e. g. Algebraic solutions of linear parti al differential equations. Make the title as informative as possible; but avoid re dundancy, and eschew the medieval practice of letting the title serve as an infl ated advertisement. A title of more than ten or twelve words is likely to be m iscopied, misquoted, distorted, and cursed.The first paragraph of the introduction should be comprehensible to any mathe matician, and it should pinpoint the location of the subject matter. The main p urpose of the introduction is to present a rough statement of the principal resul ts; include this statement as soon as it is feasible to do so, although it is som etimes well to set the stage with a preliminary paragraph. The remainder of th e introduction can discuss the connections with other results.It is sometimes useful to follow the introduction with a brief section that estab lishes notation and refers to standard sources for basic concepts and results. N ormally this section should be less than a page in length. Some authors weave this information unobtrusively into their introductions, avoiding thereby a dull section.The section following the introduction should contain the statement of one or more principal results. The rule that the statement of a theorem should precede its proof a triviality. A reader wants to know the objective of the paper, as well as the relevance of each section, as it is being read. In the case of a ma jor theorem whose proof is long, its statement can be followed by an outline of proof with references to subsequent sections for proofs of the various parts. Strive for proofs that are conceptual rather than computational. For an example of the difference, see A Mathematician’s Miscellany by J.E.Littlewood, in wh ich the contrast between barbaric and civilized proofs is beautifully and amusin gly portrayed. To achieve conceptual proofs, it is often helpful for an author t o adopt an initial attitude such as one would take when communicating mathe matics orally (as when walking with a friend). Decide how to state results wit h a minimum of symbols and how to express the ideas of the proof without computations. Then add to this framework the details needed to clinch the resul ts.Omit any computation which is routine (i.e. does not depend on unexpected tri cks). Merely indicate the starting point, describe the procedure, and state the o utcome.It is good research practice to analyze an argument by breaking it into a succe ssion of lemmas, each stated with maximum generality. It is usually bad practi ce to try to publish such an analysis, since it is likely to be long and unintere sting. The reader wants to see the path-not examine it with a microscope. A p art of the argument is worth isolating as a lemma if it is used at least twice l ater on.The rudiments of grammar are important. The few lines written on the blackbo ard during an hour’s lecture are augmented by spoken commentary, and aat t he end of the day they are washed away by a merciful janitor. Since the publ ished paper will forever speak for its author without benefit of the cleansing s ponge, careful attention to sentence structure is worthwhile. Each author must develop a suitable individual style; a few general suggestions are nevertheless a ppropriate.The barbarism called the dangling participle has recently become more prevalen t, but not less loathsome. “Differentiating both sides with respect to x, the eq uation becomes---”is wrong, because “the equation”cannot be the subject th at does the differentiation. Write instead “differentiating both sides with respec t to x, we get the equation---,”or “Differentiation of both sides with respect to x leads to the equation---”Although the notion has gained some currency, it is absurd to claim that infor mal “we”has no proper place in mathematical exposition. Strict formality is appropriate in the statement of a theorem, and casual chatting should indeed b e banished from those parts of a paper which will be printed in italics. But fif teen consecutive pages of formality are altogether foreign to the spirit of the t wentieth century, and nearly all authors who try to sustain an impersonal digni fied text of such length succeed merely in erecting elaborate monuments to slu msiness.A sentence of the form “if P,Q”can be understood. However “if P,Q,R,S,T”is not so good, even if it can be deduced from the context that the third co mma is the one that serves the role of “then.”The reader is looking at the paper to learn something, not with a desire for mental calisthenics.Vocabularypreliminary 序,小引(名)开端的,最初的(形) eschew 避免medieval 中古的，中世纪的inflated 夸张的comprehensible 可领悟的，可了解的pinpoint 准确指出（位置）weave 插入，嵌入unobtrusivcly 无妨碍地triviality 平凡琐事barbarism 野蛮，未开化portray 写真，描写clinch 使终结rudiment 初步，基础commentary 注解，说明janitor 看守房屋者sponge 海绵dangling participle 不连结分词prevalent 流行的，盛行loathsome 可恶地absurd 荒谬的banish 排除sustain 维持，继续slumsiness 粗俗，笨拙monument 纪念碑calisthenics 柔软体操，健美体操notes1. 本课文选自美国数学会出版的小册子A mamual for authors of mathematical paper的一节，本文对准备投寄英文稿件的读者值得一读。

数学专业介绍

11114210 11115010 11115090 11180110
常微分方程数值分析抽象代数数学模型
学期
课程代码 11115020 11115030 11114750
课程名称复变函数实变函数与泛函分析专业英语
学分 4.0 5.0 2.0
周学时 4 5 2
理论学时 68 85 34
数学的推理方法具有的严密性，数学的推理方法具有的严密性，对于每个懂得它的人来说都是无可争辩的。辩的。这种推理模式赋予数学以及其他科学不能比拟的精确性，他科学不能比拟的精确性，成为人类思维的一种典范，思维的一种典范，并日益渗透到其他知识领域，知识领域，此乃数学影响于人类文化的突出方面之一。的突出方面之一。与抽象性相联系的数学的另一个特点是在对宇宙世界和人类社会的探索中追求最大限度的一般性这种模式，索中追求最大限度的一般性这种模式，特别是一般性算法的倾向。特别是一般性算法的倾向。
学分 2.0
周学时 1
理论学时 17
实验学时
归口单位政社学院计科学院计科学院计科学院计科学院计科学院计科学院计科学院政社学院计科学院计科学院
课程 11177670
2.0
2
34
11177690 11177730 11178180 11178420 11177700 11179550 14100080 八 35102040 35101040
4.0 3.0 4.0 3.0 3.0 2.0 2.0 4.0 6.0
4 3 4 3 3 2 1 *
68 51 68 51 51 34 17
七
专业选修
通识必修实践必修

大学专业详解：理学（数学类）

⼀专业详解 070101　数学与应⽤数学培养⽬标：本专业培养掌握数学科学的基本理论与基本⽅法，具备运⽤数学知识、使⽤计算机解决实际问题的能⼒，受到科学研究的初步训练，能在科技、教育和经济部门从事研究、教学⼯作或在⽣产经营及管理部门从事实际应⽤、开发研究和管理⼯作的⾼级专门⼈才。

培养要求：本专业学⽣主要学习数学和应⽤数学的基础理论、基本⽅法，受到数学模型、计算机和数学软件⽅⾯的基本训练，具有较好的科学素养，初步具备科学研究、教学、解决实际问题及开发软件等⽅⾯的基本能⼒。

毕业⽣应获得的知识与能⼒： 1.具有扎实的数学基础，受到⽐较严格的科学思维训练，初步掌握数学科学的思想⽅法； 2.具有应⽤数学知识去解决实际问题，特别是建⽴数学模型的初步能⼒，了解某⼀应⽤领域的基本知识； 3.能熟练使⽤计算机(包括常⽤语⾔、⼯具及⼀些数学软件)，具有编写简单应⽤程序的能⼒； 4.了解国家科学技术等有关政策和法规； 5.了解数学科学的某些新发展和应⽤前景； 6.有较强的语⾔表达能⼒，掌握资料查询、⽂献检索及运⽤现代信息技术获取相关信息的基本⽅法，具有⼀定的科学研究和教学能⼒。

主要课程：分析学、代数学、⼏何学、概率论、物理学、数学模型/数学实验、计算机基础、数值⽅法、数学史等，以及根据应⽤⽅向选择的基本课程修业年限：四年授予学位：理学学⼠ 070102　信息与计算科学培养⽬标：本专业培养有良好的数学素养，掌握信息科学和计算科学的基本理论和⽅法，受到科学研究的初步训练，能运⽤所学知识和熟练的计算机技能解决实际问题，能在科技、教育和经济部门从事研究、教学和应⽤开发和管理⼯作的⾼级专门⼈才。

培养要求：本专业学⽣主要学习信息科学和计算科学的基本理论、基本知识和基本⽅法，打好数学基础，受到较扎实的计算机训练，初步具备在信息科学与计算科学领域从事科学研究、解决实际问题及设计开发有关软件的能⼒。

毕业⽣应获得的知识与能⼒： 1.具有扎实的数学基础，掌握信息科学和/或计算科学的基本理论和基本知识； 2.能熟练使⽤计算机(包括常⽤语⾔、⼯具及⼀些专⽤软件)，具有基本的算法分析、设计能⼒和较强的编程能⼒； 3.了解某个应⽤领域，能运⽤所学的理论、⽅法和技能解决某些科研或⽣产中的实际课题； 4.对信息科学与计算科学理论、技术及应⽤的新发展有所了解； 5.掌握⽂献检索、资料查询的基本⽅法，具有⼀定的科学研究和软件开发能⼒。

数学专业英语

第一章数学专业英语的阅读和翻译初级阶段1.1 数学专业英语的基本特点一注意对客观事实与真理的描述：1. 语句事态的使用上常用一般现在时例 An equation is a statement of the equality between two equal numbers or number symbols.2. 被动语态出现频率高“It is …”句型也使用得多例 It is cleat that any function defined for all positive real x may be used to construct a sequence by restricting x to take only integer values.3. 主动语态句型也多数用于强调事实，而不是强调行为发出者及其情感例 1：Given ε> 0，there exits a number N>0. such that ε<-a a n for all n ≥N 例 2：Since h(x) is harmonic on a neighborhood of B(a,r), we have)()()(a h x d x h B =⎰∂σ“we have ” =one has 可省略译为 “可以得出”什么结论二科学内容的完整性与表达形式的精炼性要求三数学的专业性十分典型1、有的概念可能有几个同义词如“计算”有：count calculate calculationcalculus compute computation2、同一词根的次和词组很多，如：Integrability 可积性 integrable 可积的 integral 积分，积分的，整数的 integral calculus 积分学 integralization 整化 integrate 积分3、半专业词汇多是出现频率高如:function 函数functional 泛函power 幂set 假定，令4、数学词汇具有自己的特点（不易记忆）5、表示条件，推理根据的句型相对固定如(1)用if，when, as为连接的条件从句例：The function f(x) approaches infinity as x tends to zero.(2)用with 短语表示条件或补充条件例：Suppose D is an open set with its closure in G.(3)用such that 为连接词的从句表示条件或补充条件例：Suppose f(x) is a function on a domain D such that ︳f(x) ︳< M for all x ∈D，when M is a constant.Such that =satisfying(4)虚拟语气表示条件.(5)用特殊动词，如：suppose, let, set, assume等表示假设.(6)表示推理的根据常用“by”短语，也用“according to”例：By lemma 2we have x≥y6、形成了一批数学特殊记号和表达式(1)充分必要条件The sufficient and necessary condition when and only when =if and only =iff(2)表示事先任意取定的量例：对任意的ε﹥0 即 For any number ε﹥0,或Given ε﹥01.2 数学专业英语的阅读和翻译一、翻译技巧1、词义的选择及引申例 1.A take its value 3 take value “取值”2.Two and three make fiveMake “制造”引申为“等于”3.Year after year and century after century the moon goes through itscycles of changes.月亮的盈亏变化，一年又一年，一世纪又一世纪，周而复始。

大学高数英语教材pdf

大学高数英语教材pdf大学高等数学是大部分高校理工科专业的基础课程之一，也是一门重要的学科。

对于学习者来说，拥有一本好的教材是提高学习效果的关键之一。

而对于英语学习者来说，拥有一本英语教材也是学习高数的必备工具。

虽然市面上有很多英文版的教材供选择，但其中不乏质量参差不齐的版本。

因此，寻找一本好的大学高数英语教材PDF版本变得至关重要。

下面将介绍一些值得推荐的大学高数英语教材PDF版本。

第一本推荐的教材是《高等数学（英文版）》。

这本教材由中国高等教育出版社出版，作者是Sun Chengyong等。

该教材综合了国内外大学高数教学经验，并配以丰富的例题和习题，有助于学生提高数学思维和解题能力。

该教材的PDF版通过网络可以免费下载，对于有网络条件的学生来说非常方便。

第二本推荐的教材是《微积分》。

该教材是由翻译组根据国外经典教材进行翻译，并经过多年的使用和修订，已成为国内大部分高校微积分教学的主要教材之一。

该教材PDF版本提供了全书的完整内容，包括理论知识、定理证明、例题解析和习题答案等。

对于希望系统学习微积分知识的学生来说，该教材是不可多得的资源。

第三本推荐的教材是《线性代数与解析几何》。

该教材由清华大学出版社出版，作者是张宇等。

该教材的PDF版本结合了线性代数和解析几何的相关内容，系统地介绍了向量空间、线性方程组、矩阵理论等重要知识点，并通过大量的例题和练习题来帮助学生巩固所学内容。

该教材在国内有较高的知名度和使用率，其PDF版本广泛流传于网络。

第四本推荐的教材是《概率统计与随机过程》。

该教材由北京科技大学出版社出版，作者是徐小湛等。

该教材PDF版本完整地涵盖了概率论、数理统计和随机过程三个方面的内容，以精简的篇幅准确地讲解了相关的理论和方法，并拥有大量的应用实例和习题，对于学习者来说非常实用。

除了上述推荐的教材外，还有其他一些大学高数英语教材的PDF版本可以在网络中找到。

学生可以根据自己的需求和学习风格选择适合自己的教材。

高等数学上英语教材

高等数学上英语教材高等数学在英语教材中的地位高等数学是大学数学中的核心课程之一，它在英语教材中占据着重要的地位。

作为一门高级学科，高等数学的教材内容涵盖了广泛的数学知识和技巧，对于培养学生的逻辑思维能力和解决问题的能力至关重要。

本文将通过分析高等数学在英语教材中的作用和表现形式，探讨其在学生学习中的价值和意义。

一、高等数学与英语教材的关系高等数学是一门抽象性强、逻辑性严密的学科，与英语教材有着紧密的关系。

在学习高等数学过程中，不仅需要掌握数学的概念和定理，还需要理解和运用数学术语和符号。

而这些数学术语和符号在英语教材中有着固定的英文表达方式，因此，高等数学的教材内容也体现了与英语的交叉。

二、高等数学在英语教材中的呈现方式1. 翻译与解释在高等数学的教材中，会出现许多英文术语和符号，这些术语和符号需要翻译成中文并加以解释。

例如，在介绍微积分概念时，会涉及到导数和积分等英文表达。

教材中往往会给出这些术语的中文翻译和详细解释，以帮助学生理解和掌握相关数学知识。

2. 实例与案例为了帮助学生更好地理解和应用高等数学知识，英语教材中通常会给出一些实例和案例。

这些实例和案例旨在通过具体的问题和情境，引导学生运用所学的数学方法和理论进行解决。

这种实践性的学习方式可以提高学生的数学思维能力和解决问题的能力，同时也加深了学生对高等数学的理解。

3. 启发与拓展英语教材在呈现高等数学内容时，还会引导学生思考和探索。

通过提出一些有趣的问题或开放性的讨论主题，激发学生的兴趣，并促使他们在高等数学领域进行进一步的思考和研究。

这种启发式的教学方式可以培养学生的自主学习能力和创新精神，提升他们在高等数学上的理解和应用能力。

三、高等数学在英语教材中的价值和意义1. 提高数学学习效果高等数学作为一门复杂的学科，学生在学习过程中难免会遇到各种困难和挑战。

而通过英语教材中的学习，学生既可以掌握数学的专业知识，又可以提高英语水平。

这种综合性的学习方式可以增强学生对高等数学的理解和记忆，提高他们的学习效果。