英语南方凤凰台答案一轮复习
凤凰英语第一册复习题 带答案 高职英语
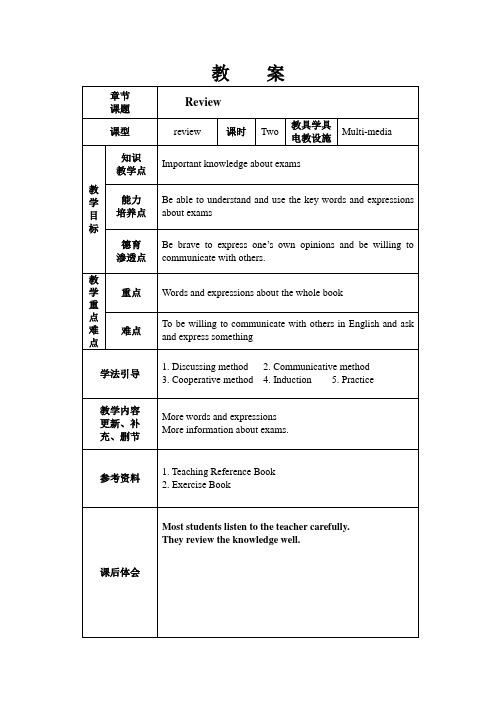
Learn and do 13′
Let Ss do the exercises
the exercises.
1. I think Jean is _______ person I’ve ever met.
A. nice
B. nicer
C. the nicest D.
more nice
2. The Summer Palace is one of _______ places in China. Discuss and 20′
learned this tern
points.
Notes:
1. subway ( )
A. 小道 B. 航道 C. 地铁 D. 公交
2. quiet ( )
A. 安静的 B. 十分 C. 夸奖 D. 快的
3. square ( )
Read, practice
教与学互动设计
教师活动内容
学生活动内容 时间
Summary
1. key words and expressions 2. reading
Assignment
1. do their book exercises 2. Preview the learned units.
3′ Listen and take notes.
Blackboard Design
Review
1. temperature presents memory 1. decided 2. accept 3. however 1. online 2. recent 3. through
1. in the middle 2. two or three times a day 3. be tired of
江西省抚州市2024学年高考英语试题一轮复习高中总复习含解析

江西省抚州市2024学年高考英语试题一轮复习高中总复习注意事项:1.答题前,考生先将自己的姓名、准考证号填写清楚,将条形码准确粘贴在考生信息条形码粘贴区。
2.选择题必须使用2B铅笔填涂;非选择题必须使用0.5毫米黑色字迹的签字笔书写,字体工整、笔迹清楚。
3.请按照题号顺序在各题目的答题区域内作答,超出答题区域书写的答案无效;在草稿纸、试题卷上答题无效。
4.保持卡面清洁,不要折叠,不要弄破、弄皱,不准使用涂改液、修正带、刮纸刀。
第一部分(共20小题,每小题1.5分,满分30分)1.—Hi, Tom, have you checked the account yet?—Certainly, I ________ it five times even though you told me to do it twice.A.checked B.will have checkedC.have been checking D.had checked2.I’d never wondered before whether or not he was kid-friendly. With one glance, I quickly that he probably wasn’t. A.agreed B.reportedC.explained D.decided3.It is so difficult a question_______ none of the kids could work it out.A.as B.that C.which D.X4.— Can you do me a favour, Mr. Smith? My car ______ start.— No problem. Y ou can count on it.A.won’t B.mustn’tC.needn’t D.shouldn’t5.—Got your driving license?—No. I too busy to have enough practice, so I didn’t take the driving test last week.A.was B.amC.have been D.had been6.Top graduates from universities are ________ by major companies.A.chased B.registeredC.offered D.compromised7.---Can you tell us your ________ for happiness and a long life?---Living every day to the fullest,definitely.A.recipe B.effortC.content D.demand8.________ on February 5, 2019, the Wandering Earth soon became a great hit.A.Releasing B.Being releasedC.Released D.To release9.It’s _______ for people to blame traffic jams, the cost of gas and the great speed of modern life.A.reasonable B.availableC.accurate D.cautious10.—Our team has just narrowly won the game. I feel so relieved now.—__________!A.Cheer up B.Y ou betC.What a pity D.Well done11.语音知识(共5小题;每小题1分,满分5分)从A、B、C、D四个选项中,找出其划线部分与所给单词的划线部分读音相同的选项。
2023高考一轮总复习金榜题名英语课时作业答案

2023高考一轮总复习金榜题名英语课时作业答案1、17.Joe is a good student and he is busy ______ his studies every day. [单选题] *A.inB.with(正确答案)C.byD.for2、Helen is new here, so we know _______ about her. [单选题] *A. somethingB. anythingC. everythingD. nothing(正确答案)3、If you get _______, you can have some bread on the table. [单选题] *A. happyB. hungry(正确答案)C. worriedD. sad4、38.These workers ___________ this bridge since one year ago. [单选题] * A.buildB.are buildingC.have built (正确答案)D.built5、My father?is _______ flowers. [单选题] *A. busy watering(正确答案)B. busy waterC. busy with wateringD. busy with water6、The students _____ outdoors when the visitors arrived. [单选题] *A. were playing(正确答案)B. have playedC. would playD. could play7、We have made a _______ tour plan to Sydney. [单选题] *A. two dayB. two daysC. two-day(正确答案)D. two-days8、95.-Dad, can we walk? ? ? ? ? ? ?the road now?-No,we? ? ? ? ? ? ? ? ? ? . We have to wait until the light turns green. [单选题] *A.across, needn’tB.across, mustn’t(正确答案)C.though, can’tD.through, mustn't9、Could you tell me _____ to fly from Chicago to New York? [单选题] *A.it costs how muchB. how much does it costC. how much costs itD.how much it costs(正确答案)10、Jim is a(n) _______. He is very careful and likes to work with numbers. [单选题] *A. secretaryB. tour guideC. accountant(正确答案)D. English teacher11、Wang Dong usually gets up at 6:00 _______ he can catch the early school bus. [单选题] *A. as ifB. so that(正确答案)C. untilD. after12、54.—________?—Yes, please. I'd like some beef. [单选题] *A.What do you wantB.May I try it onC.Can I help you(正确答案)D.What else do you want13、Jack would rather spend time complaining than_____the problem by himself. [单选题] *A.solve(正确答案)B.solvedC.solvesD.to solve14、If you want to be successful one day, you have to seize every _______ to realize your dream.[单选题] *A. changeB. chance(正确答案)C. chairD. check15、95--Where and when _______ you _______ it? [单选题] *A. did; buy(正确答案)B. do; buyC. have; boughtD. will; buy16、8.Turn right ________ Danba Road and walk ________ the road, then you will findMeilong Middle school. [单选题] *A.in...alongB.into...along (正确答案)C.in...onD.into...on17、I often _______ music from the Internet. [单选题] *A. download(正确答案)B. spendC. saveD. read18、He always did well at school _____ having to do part-time jobs every now and then. [单选题] *A despite ofB. in spite of(正确答案)C. regardless ofD in case of19、Is there ____ for one more in the car? [单选题] *A. seatB. situationC. positionD. room(正确答案)20、( )Keep quiet, please. It’s ________ noisy here. [单选题] *A. many tooB. too manyC. too muchD. much too(正确答案)21、Having stayed in the United States for more than ten years, he got an American()[单选题] *A. speechB. accent(正确答案)C. voiceD. sound22、He can’t meet his friends tonight because he _______ do homework. [单选题] *A. has to(正确答案)B. needC. have toD. don’t have to23、Mary _______ a small gift yesterday, but she didn’t _______ it. [单选题] *A. accepted; receiveB. received; accept(正确答案)C. receives; acceptedD. accepts; received24、Ships can carry more goods than _____ means of transport. [单选题] *A. the otherB. anotherC. any other(正确答案)D. any25、Bliss, who worked in an information centre, began to work on the book in 1 [单选题] *A. 策划B. 上班C. 写作(正确答案)D. 销售26、--What would you like to say to your _______ before leaving school?--I’d like to say"Thank you very much!" [单选题] *A. workersB. nursesC. waitersD. teachers(正确答案)27、He has made a lot of films, but ____ good ones. [单选题] *A. anyB. someC. few(正确答案)D. many28、Everyone knows that the sun _______ in the east. [单选题] *A. fallsB. rises(正确答案)C. staysD. lives29、David ______ at home when I called at seven o’clock yesterday evening. ()[单选题] *A. didn’tB. doesn’tC. wasn’t(正确答案)D. isn’t30、My English teacher has given us some _______ on how to study English well. [单选题] *A. storiesB. suggestions(正确答案)C. messagesD. practice。
新高考英语一轮复习专题三动词练习含答案

专题三动词综合提升练1.Butterfly Lovers combines Chinese and Western musical elements:it(play) on Western instruments such as the violin,but more significantly,much of the music has its roots in Chinese Yue Opera.(译林XB1U2)2.As Helen's knowledge and vocabulary(expand),she asked more and more questions.(北师大XB2U5)3.Even if news reports(write)from basically the same perspective,they may contradict each other in terms of factual details,as events in the real world are usually complicated and constantly changing.(译林XB2U1)4.It was the first time Helen(understand)such a complex word—a word for something she couldn't touch.(北师大XB2U5)5.He left England on the ship,the Beagle,in1831.The journey gave him the chance(study)various living things in their natural environments.(外研XB1U5)6.The more we like the brand ambassador,the more we(attract)to buy the product.(译林XB2U1)7.The young man in question,Charles Darwin,was a geologist and naturalist, (fascinate)by rocks,plants and animals.(外研XB1U5)8.Today,On the Origin of Species(regard)as one of the most important works ever written.(外研XB1U5)9.Some books are just too difficult(adapt)for technical reasons,although films like The Life of Pi are changing this idea.(北师大XB2U6)10.(locate)at over5,000metres above sea level,Tanggula station is the highest railway station in the world.(外研XB1U6改)11.In a world still(face)so many problems,it is absolutely essential for us all to collaborate to create a global community with a shared future of peace and prosperity.(外研XB2U4)12.Nowadays,people eat salted duck all year round and it is estimated that tens of thousands of ducks(consume)every day in Nanjing!(译林XB1U1)13.People in ancient China began growing wild rice,(learn)how to cultivate rice in fields and improve its quality.(2024届山东青岛莱西教学质量检测)14.The Beijing Marathon(become)one of the most popular marathons in the world in the past years,attracting thousands of participants from all over the world.(2024届辽宁朝阳期中)15.It is well known that Chinese people prefer hot meals every day,and they're also used to(have)hot drinks with it.(2024届江苏泰州姜堰期中)16.A group of Northern Ireland schoolchildren(warm)the hearts of Chinese guests on a cold December day when they performed Chinese songs.(2024届山东青岛期中联考)17.Arched bridges,sometimes(call)moon bridges,are high enough in the middle for boats to get past under.(2024届河南许昌禹州高级中学月考)18.Flames in kilns(窑)around China have been burning since the Xia and Shang dynasties. Along the way,porcelain(create).(2024届河南新乡阶段测)19.Even when porcelain was broken into pieces and buried deep in mud,the cultural values(attach)to it would never disappear.(2024届河南新乡阶段测)20.She even inquired about where(purchase)the same mattress as she used in the Asian Games Village.(2024届山西吕梁第一次模拟)21.The video(post)by the researchers on social media platforms and immediately became a trending topic online.(2024届江西萍乡第一次模拟)22.The dragon is believed(control)the waters,and is also associated with the idea of the yang.(2024届福建福州闽江口协作体期中)23.During the weeklong public holiday,numerous vacationers,especially the young(long)to escape busy city life,avoided popular holiday destinations in order to enjoy some peace and quiet.(2024届福建师大附中期中)24.The lion dance(perform)during festivals and celebrations to bring good luck and prosperity to the community.(2024届福建福州闽江口协作体期中)25.Simplified characters are convenient for people(write)and their usage has become more and more popular.(2024届福建四校联考)26.According to data released by the National Health Commission,by the end of2021,China had the largest number of dogs in the world,with over12million people(bite) each year.(2024届福建厦门双十中学期中)27.Recently,reverse(反向的)tourism(emerge)as a new trend in China.(2024届福建师大附中期中)28.What makes us(trap)in worries most is that we're not getting enough sleep, less than seven hours a night.(2023江苏南京六校联考)29.It takes72steps(produce)Longquan celadon's jade-like green.30.A philosopher commented that nobody knew what(happen)to the Earth ina century's time.31.Early to bed and early to rise(make)a man healthy,happy and wise.32.The Asian Games Memories,a remarkable cultural activity,invites the public (share)their personal experiences and memories of the previous editions of the Games,and to express their expectations and wishes for the future of the Games.33.Jane can't attend the meeting at3o'clock this afternoon because she(teach)a class at that time.34.It includes the history of AI and how the technology can(apply)in areas such as facial recognition,automatic driving and public security.35.Dai Bin,chairman of the academy,said at an online conference that with more rural residents(spend)time on leisure activities,the tourism market and travel companies will embrace new growth in the future.36.I(drive)down to London when I suddenly found that I was on the wrong road.37.It is estimated that by the end of this year,the number of people using hanfu products in China will reach6.89million,with total sales(expect)to reach10.16billion yuan.38.Marty(work)really hard on his book and he thinks he'll have finished it by Friday.39.The spokesman said that the rescue workers(leave)for the small flooded village the next day.40.We have decided that the headmaster(award)the prize to those gifted students at the meeting tomorrow.41.Lucy has a great sense of humor and always keeps her colleagues(amuse) with her stories.42.Nowadays,mail,as well as cellphones,(play)a more and more important part in our daily communication.答案1.is played2.expanded3.are written4.had understood5.to study6.will be attracted7.fascinated8.is regarded9.to be adapted10.Located11.facing12.are consumed 13.learning14.has become15.having16.warmed17.called18. was created 19.attached20.to purchase21.was posted22.to control23.longing 24.is performed 25.to write26.bitten27.has emerged28.trapped29.to produce 30.would happen 31.makes32.to share33.will be teaching34.be applied35.spending36.was driving 37.expected38.has been working39.would leave/were leaving40.will award41.amused42.is playing。
2022届高考英语一轮复习题型组合训练(Day 9)(含解析)

2022届高考英语一轮复习题型组合训练(Day 9)今日训练内容:完型填空+七选五阅读2篇+短文改错2篇+书面表达一、完形填空Very few of us become fluent in another language by learning it in high school.I went to university and then moved across the country to seek a 1 job, married and raised children. I made an effort to keep the little bit of French I learned in school, but eventually I realized that this was really2 . I was quite aware that new languages are best learned when young, and that our abilities in that regard decline with3 .However, just before my 50th birthday, I 4 French classes. After I was tested to see which group I belonged to, I was placed at almost the introductory level When I looked around at my first class, I was 5 that many of the students were learning French as a third, fourth or even fifth language.Contrary to my 6 that learning a new language was impossibly difficult, there were people who learned new languages as a matter of course.While I had always thought of myself as a 7 learner, that was no longer the case. I 8 new vocabulary rather slowly.What I learned one week seemed to 9 as soon as I learned the next skill. I had to look up the same words and language structures over and over. Now, after several years of learning, I can listen to the news in French and 10 90% of it on the first try, read a novel if the language isn't too 11 and hold up my end of a conversation if it doesn't go loo fast. I've learned so much beyond grammar and vocabulary. I've met people from around the world and all walks of life who have the 12 to make fools of themselves in order to learn something new. I've been taught by patient and inspirational teachers from many comers of the world. Listening to the news as it is 13 to the people of France, I have a renewed understanding of how something can look 14 different from another viewpoint. I've learned that a language is not just a set of words, but a way of thinking. But most of all, I've learned that it is never too 15 to learn something new.1.A.demanding B.temporary C.meaningful D.popular2.A.helpful B.pointless C.worthwhile D.necessary3.A.health B.concern C.interest D.age4.A.got ready for B.got on with C.signed up for D.fell in love with 5.A.disappointed B.amazed C.annoyed D.excited6.A.agreement B.research C.assumption D.conclusion7.A.clumsy B.confident C.serious D.quick8.A.absorbed B.consulted C.created D.recognized9.A.go down B.slip away C.pay off D.build up10.A.catch B.recite C.guess D.hear11.A.changeable B.international C.difficult D.native12.A.chance B.trick C.courage D.desire13.A.announced B.sent C.returned D.presented14.A.completely B.equally C.roughly D.slightly15.A.slow B.impossible C.inconvenient D.late二、七选五阅读Swap, Don’t shop!You keep hearing about recycling, right? But it doesn't end with bottles, cans, and paper. Clothing takes a huge amount of natural resources(资源)to make, and buying loads of new clothing(or throwing out old clothing)is not healthy for the environment. So what to do with all thoseperfectly-good-but-you're-maybe-a-little-sick-of-them clothes piled on your bedroom floor? 16.. It's the best way to get rid of your used clothes, score clothes from your friends, and have a party all at the same time.A successful swap depends on the selection of clothes, the organization of the event, and, obviously, how much fun is had. It's really easy to do! Here are a few pointers.●Invite 5—10 people so you have a nice selection. 17., and there may not be enough things to choose from; more than that, and it becomes uncontrollable.●18.. They should also prepare plenty of reusable bags to carry their "new" clothes home.●Put different types of clothing on different surfaces in the room.19.. Place a few mirrors around your room so people can see how things look when they try them on. One of the ground rules of the swap should be that everyone must try on the clothes before they take them—things always look different when you put them on.●Set a starting time. Maybe you say “go,” or turn on a certain song, or whatever. 20.. And don't forget to put out some cookies and fruits. Remember, it's a party!A.Less people than that B.Hold a clothing swapC.If two people are competing D.Just keep music playing throughoutE.Donate whatever clothes are left overF.Have everyone put their clothes in the right spotsG.Tell everyone to bring clean clothes in good conditionWith so many things happening in the world and so much work to do in our daily life, we need easy ways to calm and relax ourselves. 21.Maybe the following ideas can help you feel more settled and supported.Comforting smells can signal our brains to relax and stay in the present moment. It’s easy to bring a comforting smell into your home with a lovely plant or a bunch of fresh flowers. 22.Childhood objects can put comfort right into your hands. Turn to simple items like a blanket, a soft animal toy, or a souvenir from a childhood trip. 23.If they comforted you in your younger years, they can certainly do so again.Nature might be the fundamental source of comfort. 24.Being in nature’s presence can remind you that your life, like the oceans, mountains and forests, can remain steady through a lot of changes. Step outside to see what natural scenes are waiting for you, or bring the outdoors in with a houseplant, even a little small one.25.It’s like coming home to a familiar, comforting welcome after a long journey. My comfort book is actually my collection of Cathy comics. I discovered these during my teen years, and they never fail to make me smile and bring back so many happy memories.A.It is permanent yet ever-changing.B.These might be hidden somewhere in your room.C.It can remind you what you already know or learn.D.You can also just open the window to let fresh air in.E.They are not comforting when you are under stress or discouraged.F.Sometimes comfort can be found where we don’t always think to look.G.Cozying up to books you’ve read again and again is a great comfort to you.三、短文改错Yesterday morning I go to the gardening house to learn flower arrangement with my friend Tom. Entering the Studio, I was absorbed in the various beautiful work there. After meeting the teacher, I started to arrange flowers by selecting that we liked from the garden. Then the teacher helped us to place the choosing flowers into an European-style vase. We were permit to take our finished works from home at last. Not only did the activity foster our sense of beauty, and it also aroused our interest in art. I can't wait participate in such activities again.It is known to all, everyone has his own good points and weak points. We should often learn from other to make ourselves better. In my opinion, Peter, monitor of our class, is a model student. He always listen to the teachers carefully in class and studies very hardly. He is not good on math, but he never gives it up. Busily as he is, he is always willing help others. So, she is the one who we can always turn to. In a conclusion, I think Peter is not only a good friend and sets us a great example.四、书面表达高考在即,你校学生会正在组织以“认识自我,发展自我”为主题的英语作文比赛。
2024届高考英语一轮专题复习:语法填空巩固练习(含答案)

高考英语一轮专题复习:语法知识巩固练习Oracle bones (甲骨) were believed to be first unearthed in Anyang, once called Yin, the capital of the Shang Dynasty. Villagers then had little idea of what they had found and sold the bones to drugstores as (36) __________ (tradition) Chinese medicines. The medicines gained the notice of historians. The discovery (37) __________ (follow) by a series of archaeological excavations (挖掘).Over the past 120 years, major systematic excavations have been carried (38) __________. A relic site museum has been built at the Yinxu site, (39) __________ (recognize) as a UNESCO World Heritage Site. Discovering the oracle bones and inscriptions helped explore the cultures of Xia and Shang dynasties, (40) __________ trace the origins of the Chinese civilization.The content of the inscriptions emphasizes the respect for ancestors and other core Chinese values (41) __________ have been passed on until today. It (42) __________ (appeal) to not only scholars but also those outside the circles of archaeology. Chen Nan, a professor at Tsinghua University’s Academy of Arts and Design, has energized the life of these ancient symbols by (43) __________ (feature) them in the biaoqingbao (emoticons) he developed.“They represent the (44) __________ (clue) to our c ultural lineage (传承),” Chen says. “I feel (45) __________ our responsibility to communicate about the charm of the primitive inscription with the younger generations and foreigners.”首先还是要完全熟悉题目给出的提示词。
2023版新教材高考英语一轮总复习Unit1单元主题训练新人教版选择性必修第四册(含答案)

新教材高考英语一轮总复习:UNIT 1 单元主题训练Ⅰ.阅读理解A2022·茂名模拟Catching nearly 4,000 wild butterflies with handheld nets and taking the temperature of each tiny insect must rank among the harder of scientific efforts.However, researchers have discovered significant differences in the ability of British butterflies to maintain a suitable temperature, raising fears that global heating will threaten the populations of some species.Butterflies are ectotherms-unable to generate their own body heat and require warm temperatures to fly.However, extreme temperatures can pose problems, particularly for those butterflies that must find shady habitats to regulate (调节) their body temperature.The study shows that larger, paler butterflies are best able to protect themselves from extreme temperatures, changing the angles of their reflective wings in relation to the sun to direct heat away from or on to their bodies.The surrounding temperature of the butterfly's perch (栖息处), or air temperature, was also taken, which indicated the degree to which butterflies were seeking specific locations to regulate their body temperature.Darker, large species have greater difficulty controlling their body temperature, but even they are better than “thermal specialists”, which rely on finding a spot at a specific temperature in a landscape — a “microclimate”—to control their body temperature.“After being caught in butterfly nets on British nature reserves, the 29 different species of butterflies' temperatures were taken with a fine probe.As we plan conservation measures to address the effects of climate change, it will be important to understand not only the habitat requirements of different butterfly species, but also their temperature requirements,” said Dr Ed Turner, of the University Museum of Zoology, Cambridge, who led the work.“With this new understanding of butterflies, we should be able to better manage habitats and landscapes to protect them, and in doing so we're probably also protecting other insects too.”语篇解读:本文是一篇说明文。
2023版新教材高考英语一轮总复习Unit3单元主题训练新人教版必修第一册(含答案)

2023版新教材高考英语一轮总复习:UNIT 3 单元主题训练Ⅰ.阅读理解ASingapore is a citystate in Southeast Asia.Thanks to a program, we got a chance to explore the Garden City for one day.First, we headed to the Gardens by the bay.We were attracted by the Supertrees at once.They are highrise gardens, which produce electricity using energy from the sun, collect rainwater, and more.From there, we headed to the Cloud Forest, which I found most amazing in this Garden City.In hot and wet Singapore, this is a great place to cool off. Inside, a large waterfall comes down the side of the “cloud mountain”.This intricate structure (错综复杂的建筑) is divided into three levels and contains plants from different places.Visitors are free to walk around and learn more about all of the plants.Our journey then led us to a theater inside the Cloud Forest where we watched a tenminute film.I was totally taken aback by what I saw.I had never realized that our planet would change so greatly when the temperature increased by just five degrees.Then we headed back outside and continued on our walk.Between two of the larger Supertrees, you'll find the Skyway.Visitors can head to the top to enjoy a fantastic bird's eye view of the city.Next, we made our way to the Singapore River, where we saw a boat going by.In search of food, we headed into the Marina Bay Sands.It has what has to be the best food court I've ever eaten in, with delicious food from all over the world.We ordered different kinds of dishes, which were all tasty.Needing to walk off that huge lunch, we walked from one side of the river to the other.On the other side, we found Merlion, the symbol of Singapore.Water flows out of the mouth of this amazing creation all day long.While walking along the riverside and enjoying the beautiful view,I couldn't help but wish we had more time to explore this Garden City.语篇解读:本文是记叙文。
英语南方凤凰台答案一轮复习

江苏高考总复习一轮配套检测与评估英语详解详析Module 1Unit 1 School life一、1. show respect for 2. was devoted to3. earn my living4. was donated to5. make good use of 6 informed of7. to approve of 8. was charged with9. graduated from 10. look back on二、11. next to; whose 12. less; than13. took me; to read 14. During, e-mail; for free15. give up; that三、16.I used to go to school by bike, but now I am used to walking to school.17.I wasn’t informed of the decision until it was too late18. You can e-mail your friends for free at the school library.19. This is the old man who donated books lo the reading room20. Our club "Teenagers Today' is literary club that was started by our Chinese teacher Mr.Zhang四、根据句意可知第一空是“有经验的老师”;时间状语是过去的一段时间,所以第二空用不定式的完成式。
22.A 句意:——我认为他积极参与社会工作。
——在某种程度上我同意你的说法。
maway意为“在某种程度上,在某一点上”。
23.D考查动词词义辨析。
achieve the goals:实现目标;acqire:取得,获得;finish:结束;conclude,得出结论。
2023届高三一轮复习联考(一)全国卷英语试卷(解析版)

2023届高三一轮复习联考(一)全国卷英语试卷注意事项:1.答卷前,考生务必将自己的姓名、考生号等填写在答题卡上。
2.回答选择题时,选出每小题答案后,用铅笔把答题卡上对应题目的答案标号涂黑。
如需改动用橡皮擦干净后,再选涂其他答案标号。
回答非选择题时,将答案写在答题卡上。
写在本试卷上无效。
3.考试结束后,将本试卷和答题卡一并交回。
考试时间为120分钟满分150分第一部分听力(共两节,满分30分)做题时,先将答案标在试卷上。
录音内容结束后,你将有两分钟的时间将试卷上的答案转涂到答题卡上。
第一节(共5小题;每小题1.5分,满分7.5分)听下面5段对话。
每段对话后有一个小题,从题中所给的ABC三个选项中选出最佳选项,并标在试卷的相应位置。
听完每段对话后,你都有10秒钟的时间来回答有关小题和阅读下一小题。
每段对话仅读一遍。
1. What does the woman mean?A. She admires those who go camping.B.She dislikes going camping.C. She believes going camping is enjoyable.2. Where does the conversation most probably take place?A. Outside a theater.B. Inside a stadium.C. Beside a bookstore.3. What color is the skirt the woman is trying on?A. Yellow.B. OrangeC. Blue.4. What do we know about John?A.He likes doing sports.B.He rides a bicycle to school.C. He plans to read novels.5. How long has Henry worked for the organization?A. 3 years.B. 12 years.C. 15 years.第二节(共15小题:每小题15分,满分22.5分)听下面5段对话或独白。
高考英语一轮复习必修第一册UNIT5 LANGUAGES AROUND THE WORLD练习含答案

UNIT 5LANGUAGES AROUND THEWORLDⅠ.完形填空(2024年湖南省三湘名校教育联盟第一次大联考)My dad was a serious amateur sailor. He and his friend Chuck 1the North American Championship,sailing the beautiful wooden Dragons. One year they won the 2to go to the Olympics.Dad didn’t become 3overnight. He had to work at his skill. As a kid,that first summer he had his own small sailboat,he 4in all the races in the bay and came in last every time.At the end of the season,when awards were being 5,something happened. An elderly lady,who had watched Dad’s slow but steady 6,stepped out of her house. She generously 7 a prize made especially for him. “The Hope Cup,”she said. No other award could match the promise of hope —a quality fixed in my dad and 8us.As a parent,he was a better sailing 9. How well I remember him taking me out in our boat,the wind still 10,the water smooth in the morning hours. “Where do you feel the wind coming from?”he asked,“Look at the flags on the beach and they’ll show the 11in which it is blowing. However,every good 12learns how to feel it.”“Now,”he said,“look at the sail. If the sail 13back and forth,I needed to pull in it. If the wind was behind us,I was to let out the sail.”Since then,sailing has been 14to me. I’ve learned that we can sail 15as long as we keep track of the wind.1.A.lost B.gainedC.challengedD.changed2.A.opportunity B.gameC.fameD.trust3.A.helpful B.cheerfulC.successfulD.wealthy4.A.existed petedC.majoredD.succeeded5.A.given out B.made upC.sent awayD.taken down6.A.behaviour B.smileC.gestureD.progress7.A.reached B.offeredC.handedD.transformed8.A.left behind B.kept up withC.passed on toD.put forward to9.A.instructorB.playerC.guardD.lifter10.A.quiet B.tightC.softD.fierce11.A.time B.wayC.strengthD.direction12.A.swimmer B.sailorC.authorD.learner13.A.turned B.floatedC.shookD.fell14.A.impossible B.unbelievableC.valuelessD.effortless15.A.smoothly B.happilyC.wildlyD.aimlessly【语篇解读】本文是一篇记叙文。
2022新高考南方凤凰台英语答案

2022新高考南方凤凰台英语答案第一部分听力(共两节,满分30分)做题时,先将答案标在试卷上。
录音内容结束后,你将有两分钟的时间将试卷上的答案转涂到答题卡上。
第一节(共5小题:每小题1.5分,满分7.5分)听下面5段对话。
每段对话后有一个小题,从题中所给的A、B、C三个选项中选出最佳选项。
听完每段对话后,你都有10秒钟的时间来回答有关小题和阅读下一小题。
每段对话仅读一遍。
例:How much is the shirt?A. £19.15.B.£9.18.C. £9.15.答案是C。
1.What is the man doing?A. Asking the way.B.Giving directions.C. Correcting a mistake.2.What dress size does the woman want?A.8.B.10.C.12.3.What is the woman likely to do?A. Make a phone call.B. Handle the problem.C. Have a rest.4.Which tour does the man seem to be interested in?A. The evening tour.B. The half-day tour.C.The full-day tour.5.Where are the speakers?A.At a canteen.B.At a clinic.C.At a bank.第二节(共15小题,每小题1.5分,满分22.5分)听下面5段对话或独白。
每段对话或独白后有几个小题,从题中所给的A、B、C三个选项中选出最佳选项。
听每段对话或独白前,你将有时间阅读各个小题,每小题5秒钟;听完后,各小题将给出5秒钟的作答时间。
每段对话或独白读两遍。
听第6段材料,回答第6、7题。
《南方凤凰台》2020江苏高考总复习 一轮复习导学案

第一章集合与常用逻辑用语第1课集合的概念与运算激活思维1. (必修1P7练习1改编)用列举法表示集合{x|x2-3x+2=0}为________.2. (必修1P9练习1改编)集合A={x|0≤x<3且x∈N}的真子集个数是________.3. (必修1P19第4题改编)若集合A={0,1,2,3,4,5},B={-1,0,1,6},则A∩B=________.4.(必修1P17第6题改编)已知集合A=[1,4),B=(-∞,a),A⊆B,那么实数a的取值范围为________.5. (必修1P14习题10改编)设集合A={4,5,7,9},B={3,4,7,8,9},全集U=A∪B,则集合∁U(A∩B)中的元素共有________个.知识梳理1.集合的概念(1) 一定范围内某些________、________对象的全体构成一个______,集合中的每一个对象称为该集合的________.(2) 集合中元素的三个特性:________、________、________.(3) 集合的表示方法:________、________、________等.(4) 自然数集记作________,正整数集记作____________或__________,整数集记作__________,有理数集记作________,实数集记作________,复数集记作________.2.两类关系(1) 元素与集合的关系,用______或______表示.(2) 集合与集合的关系,用________、________或________表示.3.集合的运算(1) 交集:由所有属于集合A且属于集合B的元素构成的集合,称为A与B的________,记作______________,即A∩B=____________.(2) 并集:由所有属于集合A或者属于集合B的元素构成的集合,称为A与B的________,记作________,即A∪B=____________.(3) 补集:设A⊆S,由S中不属于A的所有元素组成的集合称为S的子集A的________,记作________,即∁S A=____________.4.常见结论(1) ∅⊆A,A∪B=B∪A,A⊆A∪B,A∩B⊆A.(2) A∩B=A⇔A⊆B,A∪B=A⇔B⊆A.(3) ∁U(A∩B)=(∁U A)∪(∁U B),∁U(A∪B)=(∁U A)∩(∁U B).课堂导学,__集合间的基本运算)(2017·江苏卷)已知集合A={1,2},B={a,a2+3}.若A∩B={1},则实数a的值为________.【高频考点·题组强化】1. (2018·江苏卷)已知集合A={0,1,2,8},B={-1,1,6,8},那么A∩B=________.2. (2018·南京三模)若集合A={x|x2+x-6=0},B={x|x2-4=0},则A∪B=________.3. (2017·南通一调)设集合A={1,3},B={a+2,5},A∩B={3},则A∪B=________.4. 已知集合A={x|x2-1=0},B={-1,2,5},那么A∩B=________.5. (2018·全国卷Ⅰ改编)已知集合A={x|x2-x-2>0},那么∁R A=________.,__集合中元素的性质)(2018·全国卷Ⅱ改编)已知集合A={(x,y)|x2+y2≤3,x∈Z,y∈Z},则A中元素的个数为________.(1) 已知集合A={1,2,4},则集合B={(x,y)|x∈A,y∈A}中元素的个数为________.(2) 已知集合A={m+2,2m2+m},若3∈A,则m的值为________.,__集合间的基本关系)已知集合A={x|-2≤x≤5},B={x|m+1≤x≤2m-1}.(1) 若B⊆A,求实数m 的取值范围;(2) 当x∈R时,不存在元素x使得x∈A与x∈B同时成立,求实数m的取值范围.(2018·杭州模拟)已知集合A={x|1≤x<5},B={x|-a<x≤a+3},若B⊆(A∩B),则实数a的取值范围为________.(2018·南京联考)已知集合A={x|x2-4x-5≤0},B={x|2x-6≥0},M=A∩B.(1) 求集合M;(2) 已知集合C={x|a-1≤x≤7-a,a∈R},若M∩C=M,求实数a的取值范围.课堂评价1. (2017·苏北四市期末)已知集合A={-2,0},B={-2,3},则A∪B=________.2. (2017·扬州期末)已知集合A={x|x≤0},B={-1,0,1,2},则A∩B=________.3. (2017·北京卷改编)已知全集U=R,集合A={x|x<-2或x>2},则∁U A=________.4. (2017·全国卷Ⅱ改编)设集合A={1,2,4},B={x|x2-4x+m=0}.若A∩B={1},则B=________.5. (2018·启东中学月考)已知集合A={x|-1<x<3},B={x|-m<x<m},若B⊆A,则m的取值范围为________.,第2课四种命题和充要条件)激活思维1. (选修21P8习题1改编)命题“若a=0,则ab=0”的逆否命题是________.2.(选修21P7练习2改编)命题“若x<0,则x2>0”及其逆命题、否命题、逆否命题这四个命题中正确命题的个数为________.3. (选修21P21习题4改编)判断下列命题的真假.(填“真”或“假”)(1) 命题“在△ABC中,若AB>AC,则C>B”的否命题为__________命题.(2) 命题“若ab=0,则b=0”的逆否命题为________命题.4. (选修21P9习题4(2)改编)“sinα=sinβ”是“α=β”的________________条件.(选填“充分不必要”“必要不充分”“充要”或“既不充分也不必要”)5.(选修21P21习题7改编)函数f(x)=x2+mx+1的图象关于直线x=1 对称的充要条件是________.知识梳理1.记“若p则q”为原命题,则否命题为“__________”,逆命题为“________”,逆否命题为“__________”.其中互为逆否命题的两个命题同真假,即等价,原命题与__________等价,逆命题与________等价.因此,四种命题为真的个数只能是偶数.2.对命题“若p则q”而言,当它是真命题时,记作p⇒q,称p是q的______条件,q是p的______条件;当它是假命题时,记作p⇒/q,称p是q的________条件,q是p的________条件.3.(1) 若p⇒q,且q⇒/p,则p是q的__________条件;(2) 若p⇒/q,且q⇒p,则p是q的__________条件;(3) 若p⇒q,且q⇒p,则p是q的________条件,记作p⇔q;(4) 若p⇒/q,且q⇒/p,则p是q的__________条件.4.证明命题条件的充要性时,既要证明原命题成立(即条件的________),又要证明它的逆命题成立(即条件的________).课堂导学__四种命题及其真假判断 给出以下四个命题:①“若x +y =0,则x ,y 互为相反数”的逆命题; ②“全等三角形的面积相等”的否命题;③“若q ≤-1,则x 2+x +q =0有实数根”的逆否命题; ④若a +b 是偶数,则整数a ,b 都是偶数. 其中真命题是________.(填序号)__充要条件的判定(2017·天津卷改编)设θ∈R ,则“⎪⎪⎪⎪θ-π12<π12”是“sin θ<12”的________条件.(2018·苏州新区实验中学测试)在△ABC 中,“A ≠60°”是“cos A ≠12”的________条件.,__结合充要条件求参数)已知集合M ={x|x<-3或x>5},P ={x|(x -a)·(x -8)≤0}. (1) 求实数a 的取值范围,使它成为M ∩P ={x|5<x ≤8}的充要条件;(2) 求实数a 的一个值,使它成为M ∩P ={x|5<x ≤8}的一个充分不必要条件; (3) 求实数a 的取值范围,使它成为M ∩P ={x|5<x ≤8}的一个必要不充分条件.(2018·启东中学检测)已知集合A ={x|y =lg (4-x)},集合B ={x|x <a},若“x ∈A ”是“x ∈B ”的充分条件,则实数a 的取值范围是________.已知集合A =⎩⎨⎧⎭⎬⎫y ⎪⎪y =x 2-32x +1,x ∈⎣⎡⎦⎤34,2,B ={x|x +m 2≥1}.若p :x ∈A ,q :x ∈B ,且p 是q 的充分不必要条件,求实数m 的取值范围.,__充要条件的证明)已知a ,b ,c 都是实数,求证:方程ax 2+bx +c =0有一个正根和一个负根的充要条件是ac<0.课堂评价1. (2018·常州一中测试)命题“若α=π4,则tan α=1”的逆否命题是________.2. (2018·泰州中学调研)“a =0” 是“函数f(x)=x 3+ax 2(x ∈R )为奇函数”的________条件.3. (2019·常州武进期中)设x ∈R ,则x 3>8是|x |>2的________条件.4. (2018·南通一中测试)已知p :a ≤x ≤a +1,q :x 2-4x<0,若p 是q 的充分不必要条件,则实数a 的取值范围是________.5. 已知函数f(x)=x1+|x|+e x ,求证:“x 1+x 2>0”是“f(x 1)+f(x 2)>f(-x 1)+f(-x 2)”的充要条件.,第3课简单的逻辑联结词、全称量词与存在量词激活思维1. (选修11P13习题3改编)若命题p:2是质数;q:不等式x2-2x-3<0的解集为(-1,3),则命题“p且q”是________命题.(填“真”或“假”)2. (选修11P15例1改编)命题“∀x∈R,x2+x+1>0”的否定是____________________.3. (选修11P16习题4改编)命题“∃x∈N,x2≤0”的否定是____________.4. (选修11P21本章测试6改编)命题“对于函数f(x)=x2+ax(a∈R),存在a∈R,使得f(x)是偶函数”为__________命题.(填“真”或“假”)5. (选修11P21本章测试10改编)已知命题p:∀x∈R,sin x+cos x>m是真命题,那么实数m的取值范围是__________.知识梳理1.全称量词我们把表示________的量词称为全称量词.对应日常语言中的“一切”、“任意的”、“所有的”、“凡是”、“任给”、“对每一个”等词,用符号“∀”表示.含有______________的命题,叫作全称命题.如“对任意实数x∈M,都有p(x)成立”简记成“______________”.2.存在量词我们把表示________的量词称为存在量词.对应日常语言中的“存在一个”、“至少有一个”、“有个”、“某个”、“有些”、“有的”等词,用符号“______”表示.含有__________的命题,叫作存在性命题.“存在实数x0∈M,使p(x0)成立”简记成“________________”.3.简单逻辑联结词有____(符号为∨),____(符号为∧),____(符号为非).4. 命题的否定:“∀x ∈M ,p(x)”与“____________”互为否定.5. 复合命题的真假:对“p 且q ”而言,当p ,q 均为真时,其为______;当p ,q 中有一个为假时,其为______.对“p 或q ”而言,当p ,q 均为假时,其为______;当p ,q 中有一个为真时,其为______.当p 为真时,非p 为______;当p 为假时,非p 为______.6. 常见词语的否定如下表所示:词语 是 一定是 都是 大于 小于 词语的否定______ ________ ________________________词语且 必有一个 至少有 n 个 至多 有一个 所有 x 都成立 词语的否定____________________________________课堂导学,__判断复合命题的真假)(2018·泰州模拟)已知命题p 1:函数y =2x -2-x 在R 上为增函数,p 2:函数y =2x +2-x 在R 上为减函数,则在命题:①p 1∨p 2;②p 1∧p 2;③(非p 1)∨p 2;④p 1∧(非p 2)中,真命题是________.(填序号)(2018·常州前黄中学测试)已知命题p :∃x ∈(-∞,0),2x<3x,命题q :∀x ∈⎝⎛⎭⎫0,π2,cos x <1,则下列命题:①p ∧q ;②p ∨(非q );③(非p )∧q ;④p ∧(非q );⑤(非p )∨(非q ).其中真命题是________.(填序号), __含有一个量词的命题的否定) 写出下列命题的否定,并判断其真假.(1) p :∀x ∈R ,x 2-x +14≥0;(2) q :所有的正方形都是矩形; (3) r :有的实数没有平方根;(4) s :所有末位数字是0或5的整数都能被5整除; (5) t :菱形的对角线互相垂直平分.(2018·南通中学)命题“∃x ∈(0,+∞),ln x =x -1”的否定是________., __求参数范围问题) (2018·南通大学附中)已知命题p :“∀x ∈[1,2],x 2-a ≥0”,命题q :“∃x ∈R ,x 2+2ax +2-a =0”.若命题“p ∧q ”是真命题,则实数a 的取值范围是________.已知命题p :m ∈R ,且m +1≤0,命题q :∀x ∈R ,x 2+mx +1>0恒成立.若“p ∧q ”为假命题,则m 的取值范围是________.课堂评价1. (2018·常州一模)命题“∃x ∈[0,1],x 2-1≥0”是________命题.(填“真”或“假”)2. (2018·淮海中学)命题“∀x ∈⎝⎛⎭⎫0,π2,sin x <1”的否定是________.3. 已知命题p :∀x ∈[0,1],a ≥e x ;命题q :∃x ∈R ,x 2+4x +a =0.若命题“p ∧q ”是真命题,则实数a 的取值范围是__________.4. 已知c>0,设命题p :函数y =c x为减函数;命题q :当x ∈⎣⎡⎦⎤12,2时,函数f (x)=x +1x >1c 恒成立.如果“p ∨q ”为真命题,“p ∧q ”为假命题,则c 的取值范围是________.5. (2018·江苏省百校联盟联考)已知命题p :“∃x ∈[1,2],使x 2+2x +a ≥0”为真命题,则实数a 的取值范围是________.第二章函数与基本初等函数Ⅰ,第4课函数的概念及其表示法激活思维1. (必修1P26练习4改编)下列对应中为函数的有________.(填序号)①A=B=N*,对任意的x∈A,f:x→|x-2|;②A=R,B={y|y>0},对任意的x∈A,f:x→1x2;③A=B=R,对任意的x∈A,f:x→3x+2;④A={(x,y)|x,y∈R},B=R,对任意的(x,y)∈A,f:(x,y)→x+y.2.(必修1P31习题6改编)直线x=1和函数y=f(x)图象的交点个数为________.3. (必修1P31习题8改编)已知函数f(x)由下表给出,则f(3)=________.x 1 2 3 4f(x) -3 -2 -4 -14.(必修1P34习题7改编)已知函数f(x)=3,1,(),1,x xf xx x⎧≤=⎨->⎩若f(x)=2,则x=________.5.(必修1P36习题3改编)已知函数f(x)在[-1,2]上的图象如图所示,则f(x)的解析式为________.(第5题)知识梳理1.函数的概念设A,B是两个______的数集,如果按某个确定的________,使对于集合A中的________元素x,在集合B中都有______的元素y和它对应,那么称________为从集合A到集合B 的一个函数,记作y=f(x),x∈A.其中所有的输入值x组成的集合A叫作函数y=f(x)的________,将所有的输出值y组成的集合叫作函数y=f(x)的__________.2. 相同函数函数的定义含有三个要素,即____________、____________和____________.当函数的________及________确定之后,函数的________也就随之确定.当且仅当两个函数的__________和________都分别相同时,这两个函数才是同一个函数.3.函数的表示方法:________、________、________.4. 映射的概念一般地,设A ,B 是两个______的集合,如果按某一个确定的对应关系f ,使对于集合A 中的__________元素x ,在集合B 中都有______确定的元素y 与之对应,那么就称对应__________为从集合A 到集合B 的一个映射.课堂导学, __函数的概念判断下列对应是否为函数: (1) x →y =x 2+2x +1,x ∈R ; (2) x →y =1x ,x ≠0,x ∈R ; (3) x →y ,其中y 4=x ,x ∈R ,y ∈R .判断下列对应是否为函数: (1) x →y =12x ,x ∈R ;(2) x →2,x ∈R ;(3) x →y ,其中y 2=x ,x >0,y ∈R ;(4) x →y ,x ∈{江苏,山东,山西,江西},y ∈{南京,济南,太原,南昌}.试判断以下各组中的两个函数是否为同一函数: (1) f (x )=x 2,g (x )=3x 3; (2) f (x )=|x |x ,1,0,()1,0;x g x x ≥⎧=⎨-<⎩(3) f (x )=x ·x +1,g (x )=x 2+x ; (4) f (x )=x 2-2x -1,g (t )=t 2-2t -1.__求函数的解析式根据下列条件求各函数的解析式: (1) 若f(x +1)=2x 2+1,求f(x);(2) 若f(x)是一次函数,且f(f(x))=4x +3,求f(x); (3) 若2f(x)-f(-x)=x +1,求f(x).(1) 已知f ⎝⎛⎭⎫1x =x 2+5x ,求f(x)的解析式;(2) 已知二次函数f(x)与x 轴的两个交点的横坐标是0和-2,且f(x)的最小值是-1,函数g(x)与f(x)的图象关于原点对称,求f(x)和g(x)的解析式;(3) 已知函数y =f(x)满足f(x)=2f ⎝⎛⎭⎫1x +x(x ≠0),求f(x)的解析式., __分段函数) (2018·姜堰中学)已知函数f(x)的定义域为实数集R ,对任意的x ∈R ,f (x -90)={lg x ,x >0,-x ,x ≤0,则f (10)-f (-100)的值为________.(2018·无锡模拟)已知函数2log ,0,()31,0,x x x f x x >⎧=⎨+≤⎩则f ⎝⎛⎭⎫f ⎝⎛⎭⎫14的值是________.已知函数21,0,3()1,0.x x f x x x⎧-≥⎪⎪=⎨⎪<⎪⎩ (1) 若f (a )>a ,求实数a 的取值范围;(2) 若f (f (b ))=-2,求实数b 的值.(1)(2017·苏州暑假测试)已知实数m≠0,函数3,2,()2, 2.x m xf xx m x-≤⎧=⎨-->⎩若f(2-m)=f(2+m),则m的值为________.(2) 设函数2222,0,(),0.x x xf xx x⎧++≤=⎨->⎩若f(f(a))=2,则实数a=________.课堂评价1. 若集合A={x|1≤x≤2},B={y|1≤y≤4},有以下4个对应法则:①f:x→y=x2;②f:x→y=3x-2;③f:x→y=-x+4;④f:x→y=4-x2.其中不能构成从A到B的函数的是________.(填序号)2. (2018·响水中学)若二次函数g(x)满足g(1)=1,g(-1)=5,且图象过原点,则g(x)的解析式为________.3. 若函数5,6,()(2),6,x xf xf x x-≥⎧=⎨+<⎩则f(3)=__________.4. (2017·全国卷Ⅲ)设函数1,0,()2,0,x x x f x x +≤⎧=⎨>⎩则满足f(x)+f ⎝⎛⎭⎫x -12>1的x 的取值范围是________.5. (2018·启东中学)已知f ⎝⎛⎭⎫x +1x =x 2+1x 2,则f(x)的解析式是________., 第5课 函数的定义域与值域)激活思维1. (必修1P 25例2改编)函数f(x)=x -2+1x -3的定义域是________.2. (必修1P 93习题5改编)已知函数y =x 2-x 的定义域为{0,1,2,3},那么其值域为________.3. (必修1P 27练习7改编)函数f(x)=x 2-2x -3,x ∈[-1,2]的最大值为________.4. (必修1P 31习题3改编)函数y =2x -1的定义域是[2,5),则其值域是________.5. (必修1P 36习题13改编)已知函数f(x)=x 2的值域为{1,4},那么这样的函数有________个.知识梳理1. 函数的定义域(1) 函数的定义域是构成函数的非常重要的部分,若没有标明定义域,则认为定义域是使得函数解析式________的x 的取值范围.(2) 分式中分母应________;偶次根式中被开方数应为________,奇次根式中被开方数为一切实数;零指数幂中底数________.(3) 对数式中,真数必须________,底数必须________,含有三角函数的角要使该三角函数有意义等.(4) 实际问题中还需考虑自变量的__________,若解析式由几个部分组成,则定义域为各个部分相应集合的交集. 2. 求函数值域的主要方法(1) 函数的________________直接制约着函数的值域,对于一些比较简单的函数可直接通过______求得值域.(2) 二次函数或可转化为二次函数形式的问题,常用________求值域.(3) 分子、分母是一次函数或二次齐次式的有理函数常用__________求值域;分子、分母中含有二次项的有理函数,常用____________求值域(主要适用于定义域为R 的函数). (4) 单调函数常根据函数的________求值域.(5) 很多函数可拆配成基本不等式的形式,可利用________求值域. (6) 有些函数具有明显的几何意义,可根据________的方法求值域. (7) 只要是能求导数的函数常采用________的方法求值域 .课堂导学__求函数的定义域)(1) (2018·南通中学)函数y =1-x 22x 2-3x -2的定义域为________.(2) 函数y =x 2lg (4x +3)+(5x -4)0的定义域为________.(3) 若函数y =f(x)的定义域是[1,2 020],则函数g(x)=f (x +1)x -1的定义域是________.(4) 已知函数f(x -1)的定义域为[3,7],那么函数f(2x +1)的定义域为________.【高频考点·题组强化】1. (2017·南通、扬州、淮安、宿迁、泰州、徐州二调)函数f(x)=lg (5-x 2)的定义域是________.2. 函数y =3-2x -x 2的定义域是________.3. (2018·南师附中)函数f(x)=log 12(2x -3)的定义域是________.4. 函数f(x)=4-x 2|x +2|的定义域为________.5. 已知函数f(2x -1)的定义域为(0,2),那么f(x)的定义域为________.求函数的值域问题提出:求函数值域比求函数定义域要复杂得多,求函数值域常与求函数最值问题紧密相联,要适当注意. 函数的值域取决于定义域和对应法则,无论采取什么方法求函数的值域,都应先考虑其定义域,同时要注意结合函数图象来解决问题. 那么,求函数值域的方法有哪些呢? ● 典型示例求下列函数的值域:(1) y =3x 2-x +2,x ∈[1,3]; (2) y =3x +1x -2; (3) y =x +41-x ; (4) y =2x 2-x +12x -1⎝⎛⎭⎫x>12.【思维导图】【规范解答】(1) (配方法)因为y =3x 2-x +2=3⎝⎛⎭⎫x -162+2312, 所以函数y =3x 2-x +2在[1,3]上单调递增. 当x =1时,原函数取得最小值4; 当x =3时,原函数取得最大值26.所以函数y =3x 2-x +2(x ∈[1,3])的值域为[4,26]. (2) (分离常数法)y =3x +1x -2=3(x -2)+7x -2=3+7x -2, 因为7x -2≠0,所以3+7x -2≠3, 所以函数y =3x +1x -2的值域为{y|y ≠3}.(3) (换元法)设t =1-x ,t ≥0,则x =1-t 2,所以原函数可化为y =1-t 2+4t =-(t -2)2+5(t ≥0),所以y ≤5, 所以原函数的值域为(-∞,5].(4) (基本不等式法)y =2x 2-x +12x -1=x (2x -1)+12x -1=x +12x -1=x -12+12x -12+12, 因为x>12,所以x -12>0,所以x -12+12x -12≥2⎝⎛⎭⎫x -12·12⎝⎛⎭⎫x -12=2,当且仅当x -12=12x -12,即x =1+22时取等号.所以y ≥2+12,即原函数的值域为⎣⎡⎭⎫2+12,+∞.【精要点评】配方法、分离常数法和换元法是求函数值域的有效方法,但要注意各种方法所适用的函数形式,还要注意函数定义域的限制.换元法多用于无理函数,换元的目的是进行化归,把无理式转化为有理式来解.二次分式型函数求值域,多采用分离出整式再利用基本不等式的方法求解. ● 总结归纳(1) 求函数值域的常用方法有观察法、反解法、换元法、配方法、基本不等式法、判别式法、单调性法等.(2) 要掌握基本初等函数y =kx ,y =kx +b(k ≠0),y =ax 2+bx +c(a ≠0),y =kx (k ≠0)值域的一般方法. ● 题组强化1. 函数y =x +13x -2的值域是________. 2. 函数y =x -1-2x 的值域是________.3. 函数y =52x 2-4x +3的值域是________.4. (2018·苏州期末)函数22,0,()1,0x x f x x x ⎧≤=⎨-+>⎩的值域为________.5. 若函数y =f(x)的值域是⎣⎡⎦⎤12,3,则函数F(x)=f(x)+1f (x )的值域是________._已知函数的定义域(值域)求参数已知函数f(x)=(1-a 2)x 2+3(1-a )x +6. (1) 若f(x)的定义域为R ,求实数a 的取值范围; (2) 若f (x )的定义域为[-2,1],求实数a 的值.已知函数f (x )=x 2+4ax +2a +6. (1) 若f (x )的值域是[0,+∞),求a 的值;(2) 若函数f (x )≥0恒成立,求g (a )=2-a |a -1|的值域.课堂评价1. (2018·苏州期中)函数y =1ln (x -1)的定义域为_______________. 2. 函数f(x)=log 2(3x +1)的值域为__________.3. (2018·无锡一中)已知函数f(x)的图象如图所示,则函数g(x)=log2f(x)的定义域是________.(第3题)4. 若函数f(x)=(a -1)x 2+(a -1)x +1的定义域为R ,则实数a 的取值范围为________.5. 若函数y =x 2-3x -4的定义域为[0,m],值域为⎣⎡⎦⎤-254,-4,则实数m 的取值范围是________.,第6课函数的单调性激活思维1.(必修1P40练习8改编)下列说法中,正确的是______.(填序号)①若定义在R上的函数f(x)满足f(2)>f(1),则函数f(x)是R上的单调增函数;②若定义在R上的函数f(x)满足f(2)>f(1),则函数f(x)在R上不是单调减函数;③若定义在R上的函数f(x)在区间(-∞,0]上是单调增函数,在区间[0,+∞)上也是单调增函数,则函数f(x)在R上是单调增函数;④若定义在R上的函数f(x)在区间(-∞,0]上是单调增函数,在区间(0,+∞)上也是单调增函数,则函数f(x)在R上是单调增函数.2.(必修1P55习题8改编)函数f(x)=ln(4+3x-x2)的单调减区间是________.3.(必修1P44习题4改编)已知函数y=f(x)是定义在R上的单调减函数,那么满足f(2-a2)<f(a)的实数a的取值范围为________.4. (必修1P39例4改编)函数y=1x在区间[1,3]上的最大值是________.5.(必修1P54本章测试6改编)若函数f(x)=5x2+mx+4在区间(-∞,-1]上是减函数,在区间[-1,+∞)上是增函数,则m=________.知识梳理1.函数单调性的定义(1) 一般地,对于____________的函数f(x),如果对于属于这个区间的______两个自变量x1,x2,当________时,都有________(或都有________),那么就说f(x)在这个区间上是增函数(或减函数).(2) 如果函数y=f(x)在某个区间上是增函数(或减函数),那么就说f(x)在这个区间上具有(严格的)单调性,这个区间叫作f(x)的__________;若函数是增函数,则称该区间为________;若函数为减函数,则称该区间为________.2.复合函数的单调性对于函数y=f(u)和u=g(x),如果当x∈(a,b)时,u∈(m,n),且u=g(x)在区间(a,b)上和y =f(u)在区间(m,n)上同时具有单调性,那么复合函数y=f(g(x))在区间(a,b)上具有__________________,并且具有这样的规律:____________________________.3.函数的最值前提设函数y=f(x)的定义域为I,如果存在实数M满足:条件①对于任意的x∈I,都有f(x)≤M;②存在x∈I,使得f(x)=M①对于任意的x∈I,都有f(x)≥M;②存在x∈I,使得f(x)=M结论M为函数y=f(x)的最大值M为函数y=f(x)的最小值课堂导学__函数单调性的判断与证明求证:f(x)=e x+1e x 在(0,+∞)上是增函数.讨论函数f(x)=xx 2-1在x ∈(-1,1)上的单调性.,由函数单调性求参数范围)(2018·南通调研)已知函数,0,()(0,1)(3)4,0x a x f x a a a x a x ⎧<=>≠⎨-+≥⎩且满足对任意x 1≠x 2,都有f (x 1)-f (x 2)x 1-x 2<0成立,则实数a 的取值范围是________.【高频考点·题组强化】1. 已知函数f(x)=3-axa -1(a ≠1).若f(x)在区间(0,1]上是减函数,则实数a 的取值范围是________.2. 若f(x)=-x 2+2ax 与g(x)=ax +1在区间[1,2]上都是减函数,则实数a 的取值范围是________.3. 已知函数f(x)=x|2x -a|(a>0)在区间[2,4]上单调递减,则实数a 的值是________.4. 已知函数f(x)=ax 2-x +1在(-∞,2)上单调递减,则实数a 的取值范围是________.5. (2018·金陵中学)若定义在[-2,2]上的函数f(x)满足(x 1-x 2)[f(x 1)-f(x 2)]>0,x 1≠x 2,且f(a 2-a)>f(2a -2),则实数a 的取值范围为________.,抽象函数的单调性)已知函数f(x)对于任意的x ,y ∈R ,总有f (x )+f (y )=f (x +y ),f (1)=-23,且当x >0时,f (x )<0.(1) 求证:f (x )在R 上是减函数;(2) 求f (x )在[-3,3]上的最大值和最小值.已知函数f (x )对任意的m ,n ∈R ,都有f (m +n )=f (m )+f (n )-1,且当x >0时,恒有f (x )>1. (1) 求证:f (x )在R 上是增函数;(2) 若f (3)=4,解不等式f (a 2+a -5)<2.课堂评价 1. (2018·常州调研)函数y =x 2+x +1(x ∈R )的单调减区间是________.2. 函数y =log 0.5(x 2-5x +6)的单调增区间为________.3. (2018·北京卷)能说明“若f(x)>f(0)对任意的x ∈(0,2]都成立,则f(x)在[0,2]上是增函数”为假命题的一个函数是________.4. (2018·苏州中学)若函数f(x)=-a x +b(a>0)在⎣⎡⎦⎤12,2上的值域为⎣⎡⎦⎤12,2,则a +b =________. 5. (2017·江苏卷)已知函数f(x)=x 3-2x +e x-1e x ,其中e 是自然对数的底数.若f(a -1)+f(2a 2)≤0,则实数a 的取值范围是________.第7课 函数的奇偶性激活思维 1. (必修1P 43练习6改编)函数f(x)=x 4-1x (x 2-1)是________函数.(填“奇”“偶”或“非奇非偶”)2. (必修1P 94习题28改编)若f(x)是定义在R 上的奇函数,且当x >0时,f (x )=2x -3,则f (-2)=________.3. (必修1P 55习题8改编)若函数f(x)=(x +a)(x -4)为偶函数,则实数a =________.4. (必修1P 43习题4改编)已知函数f(x)=4x 2+bx +3a +b 是偶函数,其定义域为[a -6,2a],那么点(a ,b)的坐标为__________.5. (必修1P 54本章测试8改编)若偶函数f(x)在(-∞,-1]上是增函数,则f ⎝⎛⎭⎫-32,f(-1),f(2) 的大小顺序是________.知识梳理1. 奇、偶函数的定义对于函数f(x)定义域内的______一个x ,都有__________(或__________),则称f(x)为奇函数;对于函数f(x)的定义域内的任意一个x ,都有__________(或__________),则称f(x)为偶函数.2. 奇、偶函数的性质(1) 具有奇偶性的函数,其定义域关于______对称(也就是说,函数为奇函数或偶函数的必要条件是其定义域关于______对称).(2) 奇函数的图象关于______对称,偶函数的图象关于______对称. (3) 若奇函数的定义域包含0,则f(0)=__________.(4) 定义在(-∞,+∞)上的任意函数f(x)都可以唯一表示成一个奇函数与一个偶函数之和.课堂导学函数奇偶性的判定判断下列各函数的奇偶性:(1) f(x)=x 3-x 2x -1;(2) f(x)=x 2-1+1-x 2; (3) f(x)=|x +2|-|x -2|;(4) 22,0,(),0.x x x f x x x x ⎧+<=⎨->⎩判断下列各函数的奇偶性: (1) f(x)=(x -1)1+x 1-x ;(2) f(x)=log a (x +x 2+1)(a>0且a ≠1).,_函数奇偶性的应用) (1) (2018·连云港模拟)若函数y =f(x)是R 上的奇函数,当x <0时,f (x )=2x ,则当x >0时,f (x )=________.(2) 若函数f (x )=(),0,()(2),0x x b x f x ax x x -≥⎧=⎨+<⎩ (a ,b ∈R )为奇函数,则f (a +b )的值为________.(1) 若f (x )为定义在R 上的奇函数,当x ≥0时,f (x )=2x +2x +b (b 为常数),则 f (-1)=________.(2) 已知f (x )=ax 7-bx +2,且f (-5)=17,那么f (5)=________._函数奇偶性与单调性的综合应用问题提出:奇函数在定义域内的单调性是怎么样的呢?偶函数在定义域内的单调性又是怎么样的呢?抽象函数中,与函数单调性有关的不等式问题,又是如何去掉抽象函数中的符号“f”的呢? ● 典型示例已知函数f(x)是定义在R 上的单调函数,对任意的实数a ∈R ,f (-a )+f (a )=0恒成立,且f (-3)=2.(1) 试判断函数f (x )在R 上的单调性,并说明理由;(2) 解关于x 的不等式:f ⎝⎛⎭⎫m -x x +f (m )<0,其中m ∈R 且m >0.【思维导图】【规范解答】(1) 函数f (x )为R 上的减函数.理由如下:由题知f (x )是R 上的奇函数,所以f (0)=0.又因为f (x )是R 上的单调函数, 由f (-3)=2,f (0)<f (-3),知f (x )为R 上的减函数.(2) 由f ⎝⎛⎭⎫m -x x +f (m )<0,得f ⎝⎛⎭⎫m -x x <-f (m )=f (-m ),结合(1)得m -x x >-m ,整理得(1-m )x -mx <0. 当m >1时,不等式的解集为⎩⎨⎧⎭⎬⎫x |x >0或x <m 1-m ; 当m =1时,不等式的解集为{x |x >0}; 当0<m <1时,不等式的解集为⎩⎨⎧⎭⎬⎫x |0<x <m 1-m .【精要点评】利用函数的单调性解函数不等式要特别注意必须考虑函数的定义域,进而结合函数单调性去求不等式的解集. ● 总结归纳奇函数在关于原点对称的区间上的单调性相同,偶函数在关于原点对称的区间上的单调性相反.抽象函数中的不等式问题,核心是去掉抽象函数中的符号“f ”,除了画出草图利用数形结合思想求解外,本质是利用奇偶性和单调性.因此,若函数具有奇偶性,在研究单调性、最值或作图象等问题时,只需在非负值范围内研究即可,在负值范围内由对称性可得.● 题组强化1. 已知定义在R 上的偶函数f (x )在[0,+∞)上单调递减,且f (1)=0,则不等式f (x -2)≤0的解集是________.2. (2017·苏北四市期末)已知函数f(x)是定义在R 上的奇函数,当x >0时,f (x )=2x -3,则不等式f (x )≤-5 的解集为________.3. 已知奇函数f(x)是定义在(-3,3)上的减函数,且满足不等式f(x -3)+f(x 2-3)<0,那么x 的取值范围为________.4. (2018·苏州期中)已知奇函数f(x)在(-∞,0)上单调递减,且f(2)=0,则不等式f (x )x -1>0的解集为________.5. 已知偶函数f(x)在[0,+∞)上是增函数,如果f(ax +1)≤f(x -2)在x ∈⎣⎡⎦⎤12,1上恒成立,求实数a 的取值范围.课堂评价1. 已知f(x)=ax 2+bx 是定义在[a -1,2a]上的偶函数,那么a +b =________.2. (2017·全国卷Ⅱ)已知函数f(x)是定义在R 上的奇函数,当x ∈(-∞,0)时,f (x )=2x 3+x 2,则f(2)=________.3. 已知定义在R上的奇函数f(x)满足:当x≥0时,f(x)=log2(2+x)+(a-1)x+b(a,b为常数).若f(2)=-1,则f(-6)的值为________.4. (2018·徐州期中)已知函数f(x)=e x-e-x+1(其中e为自然对数的底数),若f(2x-1)+f(4-x2)>2,则实数x的取值范围是________.(提示:考虑g(x)=e x-e-x的性质)5. (2018·通州中学)已知函数22,0,(),0x x xf xax bx x⎧+≤=⎨+>⎩是奇函数,求a+b的值.第8课函数的图象和周期性激活思维(第1题)1. (必修1P 31练习2改编)若f(x)的图象如图所示,则f(x)=________.2. (必修1P 45习题9改编)已知函数f(x)是定义在R 上的奇函数且周期为3,若f (1)=-1,则f (2 018)=________.3. (必修1P 29练习6改编)方程|x -1|=1x 的正实数根的个数是________.4. (必修1P 87习题14改编)任取x 1,x 2∈(a ,b),且x 1≠x 2,若f ⎝⎛⎭⎫x 1+x 22>12[f(x 1)+f(x 2)],则称f(x)是(a ,b)上的凸函数.在下列图象中,为凸函数图象的是________.(填序号),①) ,②) ,③) ,④)(第4题)5. (必修1P 45习题4改编)已知定义在R 上的偶函数f (x )满足f (x +2)·f (x )=1对于x ∈R 恒成立,且f (x )>0,则f (119)=________.知识梳理1. 作函数图象有两种方法:(1) 描点法:①______;②______;③__________.运用描点法作图前,必须对图象的特征(包括图象的存在范围、大致形状、变化趋势等)做到心中有数,这样可减少列表的盲目性和连点成线的随意性,从而确保表列在关键处,线连在恰当处.(2) 图象变换法:包括______变换、______变换、______变换.2. 周期函数:对于函数y =f(x),如果存在一个非零常数T ,使得当x 取定义域内的任何值时,都有______________,那么就称函数y =f(x)为周期函数,称T 为这个函数的周期.3. 设a 为非零常数,若对f(x)定义域内的任意x ,恒有下列条件之一成立:①f(x +a)=-f(x);②f(x +a)=1f (x );③f(x +a)=-1f (x );④f(x +a)=f (x )+1f (x )-1;⑤f(x +a)=1-f (x )1+f (x );⑥f(x +a)=f(x -a),则f(x)是周期函数,2a 是它的一个周期(上述式子分母均不为零).课堂导学作函数的图象)分别画出下列函数的图象: (1) y =x +2x -1;(2) y =⎝⎛⎭⎫12|x|;(3) y =|log 2x -1|.分别画出下列函数的图象: (1) y =|lg x|;(2) y =x 2-2|x|-1.__函数图象的简单应用(2018·苏州实验中学)定义min {a ,b}=,,,,a ab b b a ≤⎧⎨<⎩已知函数f(x)=min {x ,x 2-4x +4}+4,若动直线y =m 与函数y =f(x)的图象有3个交点,则实数m 的取值范围为________.(2018·启东中学)如图,函数y =f(x)的图象是圆x 2+y 2=2上的两段弧,则不等式f(x)>f(-x)-2x 的解集是________.(变式)_函数的周期性) (1) (2018·江苏卷)已知函数f(x)满足f(x +4)=f(x)(x ∈R ),且在区间(-2,2]上,f (x )=⎩⎨⎧cosπx 2,0<x ≤2,⎪⎪⎪⎪x +12,-2<x ≤0, 则f (f (15))的值为________.(2) (2018·全国卷Ⅱ)已知f (x )是定义域为(-∞,+∞)的奇函数,且满足f (1-x )=f (1+x ).若f (1)=2,则f (1)+f (2)+f (3)+…+f (50)=________.(2018·如皋模拟)已知定义在R 上的奇函数f (x )满足f (x -4)=-f (x ),且在区间[0,2]上是增函数.若方程f (x )=m (m >0)在区间[-8,8]上有四个不同的根x 1,x 2,x 3,x 4,则x 1+x 2+x 3+x 4=________.课堂评价1. (2018·宿迁、泰州调研)已知函数f(x)=log a (x +b)(a >0且a ≠1,b ∈R )的图象如图所示,则a +b 的值是________.(第1题)2. (2017·南京三模)已知函数f(x)是定义在R 上且周期为4的偶函数.当x ∈[2,4]时,f (x )=⎪⎪⎪⎪log 4⎝⎛⎭⎫x -32,则f ⎝⎛⎭⎫12的值为________.3. 已知f(x)是定义在R 上的偶函数,且f (x +4)=f (x -2).若当x ∈[-3,0]时,f (x )= 6-x ,则f (919)=________.4. (2018·海门中学)已知函数f(x)=|x -2|+1,g(x)=kx.若方程f(x)=g(x)有两个不相等的实数根,则实数k 的取值范围是________.5. (2018·沛县中学)已知函数f(x)=⎩⎪⎨⎪⎧|log 2x|,0<x ≤2,13x 2-83x +5,x>2.若函数g(x)=f(x)-m 存在四个不同的零点,则实数m 的取值范围是________., 第9课 二次函数、幂函数激活思维1. (必修1P 54测试7改编)函数f(x)=x 2+2x -3,x ∈[0,2]的值域为________.2. (必修1P 47习题9改编)若函数y =x 2+(a +2)x +3,x ∈[a ,b]的图象关于直线x =1对称,则b =________.3. (必修1P 44习题3改编)函数2221,0,()21,0x x x f x x x x ⎧+-≥=⎨-+-<⎩的单调增区间是________.4. (必修1P 89练习3改编)若幂函数y =f(x)的图象经过点⎝⎛⎭⎫9,13,则f(25)=________.5. (必修1P 73练习3改编)已知幂函数y =(m 2-5m +7)·xm2-6在(0,+∞)上单调递增,那么实数m =________.第9-12课时内容丢失知识梳理1. 对数的相关概念(1) 对数的定义:如果a b =N(a >0,a ≠1),那么b 叫作______________,记作________. (2) 常用对数和自然对数①常用对数:以______为底N 的对数,简记为lg N ; ②自然对数:以______为底N 的对数,简记为ln N. (3) 指数式与对数式的相互转化 a b =N ⇔________(a >0,a ≠1,N >0),两个式子表示的a ,b ,N 三个数之间的关系是一样的,并且可以互化.2. 对数运算的性质(M >0,N >0,a >0,a ≠1) (1) log a (MN)=____________; (2) log a MN =____________;(3) log a M n =__________.3. 对数换底公式(N >0,a >0,a ≠1,b >0,b ≠1) log b N =____________.由换底公式可以得到:log a b =________,log an b m =________,log ab·log b c =________. 4. 几个常用的结论(N >0,a >0,a ≠1) (1) log a a =______,log a 1=______; (2) log a a N =______,a log aN =______.课堂导学__对数的计算 计算:(1) lg 14-2lg 73+lg 7-lg 18; (2) 2log 32-log 3329+log 38-5log 53; (3) 12lg 3249-43lg 8+lg 245; (4) (lg 2)2+lg 2·lg 50+lg 25.计算:⎝⎛⎭⎫lg 14-lg 25÷100-12=________.计算:lg 37+lg 70-lg 3-(lg 3)2-lg 9+1., __指数式与对数式的互化 已知实数x ,y ,z 满足3x =4y =6z >1. (1) 求证:2x +1y =2z ;(2) 试比较3x ,4y ,6z 的大小.。
2024届高考英语一轮复习课时提能练Unit4HistoryandTraditions含答案

Unit 4 History and Traditions一、阅读理解AEach April Thais celebrate their "Thai New Year" with a great water fight on the streets. People throw water at e v eryone passing by and e v en block the road so they can enter buses and "paint the faces" of all the passengers. But there are a few people who don’t wish to participate in the fun. When I first experienced this celebration, I was one of them.The first time our bus was stopped, 4 people coming into the bus intended to painte v eryone’s face. I slowly shoo k my head to say "No, thank you", but a man came from behind me and quickly painted my face. Then the other three felt inspired and added a little more "paint" to my face. I didn’t acti v ely stop them for fear that if I did, they would become more aggressi v e and throw e v en more water at me and possibly my backpack, which would damage whate v er was inside.E v en though it happened only once, emotionally it was v ery frightening because I felt so powerless. The experience caused me to think a lot about respect and moral autonomy orindi v idual judgment v s "permission" from the authorities. On this day, people of Thailand,including the police, gi v e "permission" for these acti v ities. So what about respect for theindi v idual? If someone does not want to be painted, is it OK to do it anyhow because the majority is ha v ing a good time and sincerely belie v es it is harmless fun, or because the police allow it?In my opinion, if someone does not want to ha v e something done to them,then you’re supposed to respect their decision. You can’t rely on the authorities or some group to tell you if something is right or wrong or if you should or shouldn’t do something. I belie v e this is a much healthier way of li v ing with one another in this world.每年四月,泰国人都会在街头举行大规模的"水仗"来庆祝他们的"泰国新年"。
《南方凤凰台》2020江苏高考总复习 一轮配套精练--答案

高考总复习 一轮配套精练 数学文科答案详析第一章 集合与常用逻辑用语 第1课 集合的概念与运算A 应知应会1. {2,4,5} 【解析】因为全集U ={1,2,3,4,5},A ={1,3},所以∁U A ={2,4,5}.2. {0,1} 【解析】因为A ={x||x|<2}={x|-2<x<2},B ={-2,0,1,2},所以A ∩B ={0,1}.3. {-1,0,1} 【解析】因为A ={0,1},B ={-1,0},所以A ∪B ={-1,0,1}.4. 5 【解析】A ∪B ={-1,0,1,2,7},所以集合A ∪B 中元素的个数为5.5. 0 【解析】因为A ={1,3},B ={a 2+2,3},且A ∪B ={1,2,3},所以a 2+2=2,即a =0.6. 1 【解析】因为B ⊆A ,所以a ∈A ,所以a =a ,解得a =1或a =0(舍去).7. 【解答】因为A ={x|x 2-1≤0}={x|-1≤x ≤1},B ={x|0<x ≤3},所以A ∩B ={x|0<x ≤1},A ∪B ={x|-1≤x ≤3}.又∁U A ={x |1<x ≤4},∁U B ={x |-1≤x ≤0或3<x ≤4},所以(∁U B )∩A ={x |-1≤x ≤0}. 8. 【解答】①当a<0时,A =∅,显然A ∩B =∅成立.②当a ≥0时,A ={x|2-a ≤x ≤2+a},B ={x|x ≤1或x ≥4}, 由A ∩B =∅,得⎩⎪⎨⎪⎧2-a>1,2+a<4,a ≥0,解得0≤a<1.综上所述,a 的取值范围为(-∞,1).B 巩固提升1. {2,6} 【解析】因为全集I ={1,2,3,4,5,6},集合A ={1,3,5},所以∁I A ={2,4,6},又因为B ={2,3,6},所以(∁I A)∩B ={2,6}.2. {-1,0,1} 【解析】由并集的定义可得A ∪B ={-1,0,1,2,3,4},结合交集的定义可知(A ∪B)∩C ={-1,0,1}.3. [-2,1) 【解析】由4-x 2≥0,得-2≤x ≤2,所以A ={x|-2≤x ≤2};由1-x>0,得x<1,所以B ={x|x<1},故A ∩B ={x|-2≤x<1}.4. {x|x <0} 【解析】因为集合B ={x|x <0},所以A ∩B ={x|x <0}.5. -2 【解析】因为A =B ,所以a 2=4,解得a =±2.又因为a<0,所以a =-2.6. (-∞,-1]∪[5,+∞) 【解析】因为∁U B =(-∞,0)∪[5,+∞),又A ⊆(∁U B),所以a +1≤0或a ≥5,解得a ≤-1或a ≥5.7. 【解答】(1) 由题可知⎩⎨⎧x =4,y =3,所以⎩⎪⎨⎪⎧x =16,y =3,故x +y =19.(2) 假设存在实数x ,使得B ⊆A ,则2-x =3或2-x =x.若2-x =3,则x =-1,不合题意;若2-x =x ,则x +x -2=0,解得x =1,不合题意. 故不存在实数x ,使得B ⊆A.8. 【解答】(1) 当a =0时,A ={x|0≤x ≤3},B ={x|-3≤x ≤2},所以∁R B ={x |x <-3或x >2},所以A ∪B ={x |-3≤x ≤3},A ∩(∁R B )={x |2<x ≤3}. (2) 因为A ∩B =A ,所以A ⊆B ,所以⎩⎪⎨⎪⎧a ≥-3,a +3≤2,解得-3≤a ≤-1,所以实数a 的取值范围为[-3,-1].第2课 四种命题和充要条件A 应知应会 1. 逆否命题2. ②③ 【解析】①原命题的否命题为“若a ≤b ,则a 2≤b 2”,假命题.②原命题的逆命题为“若x ,y 互为相反数,则x +y =0”,真命题.③原命题的逆否命题为“若x ≥2或x ≤-2,则x 2≥4”,真命题.3. 充分不必要 【解析】因为q :x ≤1或x ≥4,所以p 是q 的充分不必要条件.4. 0 【解析】由题意可得⎩⎪⎨⎪⎧x<1,x ≥a 或⎩⎪⎨⎪⎧x ≥1,x<a 成立的充要条件为{x|0≤x<1},所以a 的值是0.5. 3或4 【解析】由x 2-4x +n =0,得(x -2)2=4-n ,即x =2±4-n.因为n ∈N *,方程有整数解,所以n =3或4,故当n =3或4时方程有整数解.6. ⎣⎡⎦⎤-12,43 【解析】解不等式|x -m|<1,得 m -1<x<m +1.由题可得⎝⎛⎭⎫13,12⊆ (m -1,m +1),故⎩⎨⎧m -1≤13,m +1≥12且等号不同时成立,解得-12≤m ≤43.7. 【解答】由x 2-5x +6≥0,得x ≥3或x ≤2.因为命题q 为假,所以x ≤0或x ≥4.则{x|x ≥3或x ≤2}∩{x|x ≤0或x ≥4}={x|x ≤0或x ≥4}. 所以满足条件的实数x 的取值范围是(-∞,0]∪[4,+∞).8. 【解答】由x 2+x -6<0,得-3<x<2,即A =(-3,2).又由x -a>0,得x>a ,即B =(a ,+∞).因为“x ∈A ”是“x ∈B ”的充分条件,所以(-3,2)⊆(a ,+∞),所以a ≤-3.故实数a 的取值范围是(-∞,-3].B 巩固提升1. 必要不充分 【解析】由2a >2b ,解得a>b ,由“lg a>lg b”解得a>b>0,所以“2a >2b ”是“lg a>lg b”的必要不充分条件.2. 充分不必要 【解析】若存在负数λ,使得m =λn ,则m ·n =λn ·n =λn 2<0成立,所以为充分条件;当m ·n <0时,m 与n 不一定共线,所以“存在负数λ,使得m =λn ”不一定成立,所以不是必要条件.综上可知,“存在负数λ,使得m =λn ”是“m ·n <0”的充分不必要条件.3. (1,4) 【解析】当1≤x ≤2时,|f(x)-a|<2恒成立,即f(x)-2<a<f(x)+2,当1≤x ≤2时,f(x)+2的最小值是4,f(x)-2的最大值是1,所以1<a<4,故实数a 的取值范围是(1,4).4. 1,-1(答案不唯一) 【解析】使“若a>b ,则1a <1b ”为假命题,举一反例即可,只需取a =1,b =-1即可满足,所以满足条件的一组a ,b 的值为1,-1(答案不唯一).5. ①②④ 【解析】①因为Δ=4-4(-k)=4+4k>0,所以①是真命题;②其逆否命题为真,故②是真命题;③“a =±1”是“直线x -ay =0与直线x +ay =0互相垂直”的充要条件,故③是假命题;④否命题为“若xy ≠0,则x ,y 都不为零”是真命题.6. ⎣⎡⎭⎫0,12 【解析】因为p :12≤x<1,q :a<x<a +1,所以由题意可得⎩⎪⎨⎪⎧a<12,a +1≥1⇒0≤a<12.7. 【解答】因为集合A ={x|x 2-6x +8<0}={x|2<x<4},B ={x|(x -a)(x -3a)<0}.(1) 当a =0时,B =∅,不合题意.当a>0时,B ={x|a<x<3a},要满足题意,则⎩⎪⎨⎪⎧a ≤2,3a ≥4,解得43≤a ≤2.当a<0时,B ={x|3a<x<a},要满足题意,则⎩⎪⎨⎪⎧3a ≤2,a ≥4,无解.综上,实数a 的取值范围为⎣⎡⎦⎤43,2. (2) 要满足A ∩B =∅,当a>0时,B ={x|a<x<3a},则a ≥4或3a ≤2,即0<a ≤23或a ≥4.当a<0时,B ={x|3a<x<a},则a ≤2或3a ≥4,即a<0. 当a =0时,B =∅,A ∩B =∅.综上,实数a 的取值范围为⎝⎛⎦⎤-∞,23∪[4,+∞). 8. 【解答】因为命题p 是真命题,所以0<m<6,m ∈N ,①所以A =⎩⎨⎧⎭⎬⎫x ⎪⎪mx -1x <0=⎩⎨⎧⎭⎬⎫x ⎪⎪0<x <1m .由题意知B ={x |x 2-3x -4≤0}={x |-1≤x ≤4},C ={x |log 12x >1}=⎩⎨⎧⎭⎬⎫x ⎪⎪0<x <12. 因为命题q ,r 都是真命题,所以A B ,C A ,所以⎩⎨⎧1m≤4,1m >12.② 由①②得m =1.第3课 简单的逻辑联结词、全称量词与存在量词A 应知应会1. 假2. ∀x ∈R ,x 2-x +1≠03. ②③ 【解析】由不等式的性质可知,命题p 是真命题,命题q 为假命题,故①p ∧q 为假命题;②p ∨q 为真命题;③非q 为真命题,则p ∧(非q)为真命题;④非p 为假命题,则(非p)∨q 为假命题.4. [-3,0] 【解析】因为命题p “∃x ∈R ,2ax 2+ax -38>0”为假命题,所以对任意的x ∈R ,都有2ax 2+ax -38≤0.当a =0时,显然成立;当a ≠0时,a <0,且Δ=a 2+3a ≤0,所以-3≤a <0.综上,实数a 的取值范围是[-3,0].5. ⎝⎛⎦⎤12,23 【解析】命题p :关于x 的函数y =x 2-3ax +4在[1,+∞)上是增函数,即3a 2≤1,解得a ≤23.命题q :关于x 的函数y =(2a -1)x 在R 上为减函数,即 0<2a -1<1,解得12<a <1.若“p ∧q ”为真命题,则有a ≤23且12<a <1,所以12<a ≤23,即实数a 的取值范围是⎝⎛⎦⎤12,23. 6. [2,+∞) 【解析】依题意知p ,q 均为假命题.当p 是假命题时,mx 2+1>0恒成立,则有m ≥0;当q 是假命题时,则有Δ=m 2-4≥0,解得m ≤-2或m ≥2.因此由p ,q 均为假命题得⎩⎪⎨⎪⎧m ≥0,m ≤-2或m ≥2,即m ≥2.7. 【解答】由a 2x 2+ax -2=0,得(ax +2)·(ax -1)=0,所以x =-2a 或x =1a .若只有一个实数x 满足不等式x 2+2ax +2a ≤0,即Δ=(2a)2-8a =0,因为a>0,所以a =2.因为“p ∨q ”是假命题,所以p ,q 均为假命题,所以⎩⎪⎨⎪⎧⎪⎪⎪⎪-2a >1,⎪⎪⎪⎪1a >1,a ≠2,a>0,解得0<a<1.所以实数a 的取值范围是(0,1).8. 【解答】p :-1≤x ≤5.(1) 因为p 是q 的充分条件,所以[-1,5]是[1-m ,1+m]的子集,所以⎩⎪⎨⎪⎧m>0,1-m ≤-1,1+m ≥5,解得m ≥4.所以实数m 的取值范围为[4,+∞).(2) 当m =5时,q :-4≤x ≤6.由题意知p 与q 一真一假.当p 真q 假时,由⎩⎪⎨⎪⎧-1≤x ≤5,x<-4或x>6,得x ∈∅.当p 假q 真时,由⎩⎪⎨⎪⎧x<-1或x>5,-4≤x ≤6,得-4≤x<-1或5<x ≤6.所以实数x 的取值范围为[-4,-1)∪(5,6].B 巩固提升1. ④ 【解析】由指数函数图象恒过点(0,1),函数y =a x +1+1的图象是由y =a x 先向左平移1个单位长度,再向上平移1个单位长度得到,所以函数y =a x +1+1的图象恒过点(-1,2),故命题p 为真命题;命题q :直线m 与平面β的位置关系也可能是m ⊂β,故q 是假命题,所以p ∧(非q)为真命题.故④正确.2. 1 【解析】因为“∃x ∈R ,x 2+2x +m ≤0”是假命题,所以“∀x ∈R ,x 2+2x +m >0”是真命题,所以Δ=4-4m <0,解得m >1,故a 的值是1.3. ⎣⎡⎦⎤23,1 【解析】令f(x)=x 2-4ax +3a 2,根据题意可得⎩⎪⎨⎪⎧f (1)=1-4a +3a 2≤0,f (2)=4-8a +3a 2≤0,解得23≤a ≤1,所以实数a 的取值范围是⎣⎡⎦⎤23,1. 4. ①③ 【解析】①命题p 为真命题,命题q 为真命题,所以“p ∧(非q)”为假命题,故①正确;②当a =b =0时,有l 1⊥l 2,故②不正确;③正确.所以正确的结论为①③.5. ①② 【解析】对于①,由存在x ∈[0,2],使x 2-a ≥0成立,可得a ≤4,因此为充分不必要条件,故①正确;②显然正确;对于③,若“p ∧q ”为假命题,则p ,q 中至少有一个假命题,所以③错误.6. (-∞,1] 【解析】由题意知,f(x 1)min ⎝⎛⎭⎫x 1∈⎣⎡⎦⎤12,1≥g(x 2)min (x 2∈[2,3]),因为f(x)=x +4x ,x ∈⎣⎡⎦⎤12,1,所以f′(x)=1-4x 2<0,所以f(x)在⎣⎡⎦⎤12,1上单调递减,所以f(x)min =f(1)=5,又因为g(x)在[2,3]上的最小值为g(2)=4+a ,所以5≥4+a ,即a ≤1.7. 【解答】(1) 若p 为真命题,则ax 2+2x +a>0的解集为R ,则a >0且4-4a 2<0,解得a >1. 若q 为真命题,则a4≥1,即a ≥4.因为“p ∧(非q )”为真命题,所以p 为真命题且q 为假命题, 所以实数a 的取值范围是(1,4).(2) 解不等式(x -m )(x -m +2)<0,得m -2<x <m , 即A =(m -2,m ).由(1)知,B =(1,+∞).因为A ∩B =A ,则A ⊆B ,所以m -2≥1,即m ≥3. 故实数m 的取值范围为[3,+∞).8. 【解答】(1) 由题意得f′(x)=3x 2+2ax +1≥0对x ∈(-∞,+∞)恒成立,所以Δ=4a 2-12≤0,解得-3≤a ≤ 3.所以实数a 的取值范围为[-3,3].(2) 若q 真:因为g′(x)=e x -1≥0对任意的x ∈[0,+∞)恒成立, 所以g(x)在[0,+∞)上单调递增,则g(0)=a +1>0⇒a>-1. 由“p ∨q ”为真命题,“p ∧q ”为假命题知p ,q 一真一假.若p 真q 假,则⎩⎨⎧-3≤a ≤3,a ≤-1,解得a ∈[-3,-1];若p 假q 真,则⎩⎨⎧a<-3或a>3,a>-1,解得a ∈(3,+∞).综上所述,a 的取值范围为[-3,-1]∪(3,+∞).第二章 函数与基本初等函数Ⅰ 第4课 函数的概念及其表示法A 应知应会1. 0或-2 【解析】令x 2+2x +3=3,解得x =0或-2. 2.5x +1x 2 【解析】令t =1x (t ≠0),所以x =1t ,所以f(t)=1t 2+5t ,所以f(x)=5x +1x 2. 3. 13 【解析】由题意知g ⎝⎛⎭⎫13=ln 13<0,所以 g ⎝⎛⎭⎫g ⎝⎛⎭⎫13=eln 13=13. 4. ③ 【解析】①中,g(x)=x 2=|x|≠x ;②中,g(x)=(x -1)0=1(x ≠1);③中,f(x)=1(x>0),g(x)=1(x>0);④中,f(x)=x 2-9x +3=x -3(x ≠-3).故③中表示同一函数.5. 2 【解析】因为f(x)=⎩⎪⎨⎪⎧x ,x ≥1,1x ,0<x<1,2x,x ≤0,所以f(-2)=2-2=14,f ⎝⎛⎭⎫14=4,f(4)=4=2,所以f(f(f(-2)))=2.6. 1 【解析】令3x -1=-710,得x =10,所以f ⎝⎛⎭⎫-710=lg 10=1. 7. 【解答】(1) 设f(x)=ax 2+bx(a ≠0), 则a(x +1)2+b(x +1)=ax 2+bx +x +1,即ax 2+(2a +b)x +a +b =ax 2+(b +1)x +1,所以⎩⎪⎨⎪⎧2a +b =b +1,a +b =1,解得a =12,b =12.因此f(x)=12x 2+12x.(2) 由已知得⎩⎨⎧f (x )+2f ⎝⎛⎭⎫1x =2x +1,f ⎝⎛⎭⎫1x +2f (x )=2x +1,消去f ⎝⎛⎭⎫1x ,得f(x)=4+x -2x 23x =43x -23x +13. (3) 设t =2x +1(t>1),则x =2t -1,所以f(t)=lg 2t -1(t>1),故f(x)=lg 2x -1(x>1).8. 【解答】由题意知此框架的面积是由一个矩形和一个半圆组成的图形的面积,而矩形的长为2x ,宽为a ,半圆的直径为2x ,半径为x ,则有2x +2a +πx =l ,即a =l 2-π2x -x ,所以y =πx 22+(l 2-π2x -x)·2x =-⎝⎛⎭⎫2+π2x 2+lx. 根据实际意义知l 2-π2x -x>0,因为x>0,解得0<x<l2+π,即函数y =-⎝⎛⎭⎫2+π2x 2+lx 的定义域是{x|0<x<l2+π}.B 巩固提升1. 2x -1 【解析】由g(x +2)=f(x),得g(x +2)=2x +3.令t =x +2,则x =t -2,代入可得g(t)=2t -1,从而g(x)=2x -1.2. 6 【解析】当0<a<1时,a +1>1,所以f(a)=a ,f(a +1)=2(a +1-1)=2a.由f(a)=f(a +1),得a =2a ,所以a =14.此时f ⎝⎛⎭⎫1a =f(4)=2×(4-1)=6.当a ≥1时,a +1>1,所以f(a)=2(a -1),f(a +1)=2(a +1-1)=2a.由f(a)=f(a +1),得2(a -1)=2a ,无解.综上,f ⎝⎛⎭⎫1a =6.3. 9 【解析】因为f ⎝⎛⎭⎫19=log 319=-2,所以f ⎝⎛⎭⎫f ⎝⎛⎭⎫19=f(-2)=⎝⎛⎭⎫13-2=9. 4. f(x)=1516x -916x +18(x ≠0) 【解析】用1x代替x ,得3f ⎝⎛⎭⎫1x +5f(x)=3x +1, 所以⎩⎨⎧3f (x )+5⎝⎛⎭⎫1x =3x +1,①3f ⎝⎛⎭⎫1x +5f (x )=3x +1,②由①②得f(x)=1516x -916x +18(x ≠0).5. [-3,2] 【解析】由表格数据作出二次函数的草图,结合数据与图象即可发现不等式f(x)≤0的解集为[-3,2].6. (-2,1) 【解析】作出函数f(x)=⎩⎪⎨⎪⎧2x +1,x>0,0,x =0,2x -1,x<0的图象如图所示,所以f(x)是定义域为R 的奇函数也是增函数,所以不等式f (x 2-2)+f (x )<0,即f (x 2-2)<f (-x ),x 2-2<-x ,解得-2<x <1,所以原不等式的解集为(-2,1).(第6题)7. 【解答】(1) 因为0<c <1,所以c 2<c. 由f(c 2)=98,得c 3+1=98,所以c =12.(2) 由(1)得f(x)=⎩⎨⎧12x +1,0<x<12,2-4x+1,12≤x<1.当0<x <12时,12x +1>28+1,解得24<x <12;当12≤x <1时,2-4x +1>28+1,解得12≤x <58. 所以不等式的解集为⎩⎨⎧⎭⎬⎫x ⎪⎪24<x<58.8. 【解答】(1) 由题意及函数图象,得⎩⎨⎧402200+40m +n =8.4,602200+60m +n =18.6,解得m =1100,n =0,所以y =x 2200+x100(x ≥0).(2) 令x 2200+x100≤25.2,得-72≤x ≤70.因为x ≥0,所以0≤x ≤70.故行驶的最大速度是70 km /h .第5课 函数的定义域与值域A 应知应会1. {x|x>3} 【解析】要使函数有意义,则有x -3>0,所以x>3,故函数的定义域为{x|x>3}.2. [2,+∞) 【解析】要使函数f(x)有意义,则log 2x -1≥0,解得x ≥2,即函数f(x)的定义域为[2,+∞).3. {1,4} 【解析】当x =-1时,f(x)=1;当x =2时,f(x)=4,所以f(x)的值域是{1,4}.4. [0,2] 【解析】-x 2+4x =-(x -2)2+4≤4,所以0≤-x 2+4x ≤2,所以0≤2--x 2+4x ≤2,所以0≤y ≤2.5. [4,+∞) 【解析】当m =0时,不符合题意,所以⎩⎪⎨⎪⎧m>0,Δ=m 2-4m ≥0,解得m ≥4.6. [-3,+∞) 【解析】当x>1时,f(x)∈(0,1);当x ≤1时,f(x)∈[-3,+∞),所以f(x)∈[-3,+∞).7. 【解答】(1) 因为集合A 表示函数f(x)=1x +2+lg (3-x)的定义域,所以⎩⎪⎨⎪⎧x +2>0,3-x>0,即A =(-2,3),所以∁U A =(-∞,-2]∪[3,+∞).(2) 因为A ∪B =B ,所以A ⊆B ,所以a ≥3. 故实数a 的取值范围是[3,+∞). 8. 【解答】令f(x)=mx 2+x +1.(1) 由题意知f(x)≥0在R 上恒成立.①当m =0时,f (x )=x +1≥0在R 上不恒成立;②当m ≠0时,要满足题意,必有⎩⎪⎨⎪⎧m >0,Δ=1-4m ≤0,所以m ≥14.综上所述,实数m 的取值范围是⎣⎡⎭⎫14,+∞. (2) 由题意知,f (x )=mx 2+x +1能取到一切大于或等于0的实数.①当m =0时,f (x )=x +1可以取到一切大于或等于0的实数;②当m ≠0时,要满足题意,必有⎩⎪⎨⎪⎧m >0,Δ=1-4m ≥0,所以0<m ≤14.综上所述,实数m 的取值范围是⎣⎡⎦⎤0,14. B 巩固提升1. [-4,0)∪(0,1) 【解析】函数的定义域必须满足条件 ⎩⎪⎨⎪⎧x ≠0,x 2-3x +2≥0,-x 2-3x +4≥0,x 2-3x +2+-x 2-3x +4>0,解得x ∈[-4,0)∪(0,1). 2. (0,1] 【解析】由⎩⎪⎨⎪⎧2x ≥0,2x -1≥0,解得x ≥12,即函数的定义域为⎣⎡⎭⎫12,+∞,函数y =2x -2x -1=12x +2x -1,令t(x)=2x +2x -1,则t(x)在⎣⎡⎭⎫12,+∞上单调递增,当x =12时,t(x)min =1,即t(x)≥1,所以y =1t∈(0,1],即函数的值域为(0,1].3. [-5,-1] 【解析】因为1≤f(x)≤3,所以1≤f(x +3)≤3,所以-6≤-2f(x +3)≤-2,所以-5≤F(x)≤-1.4. [0,1] 【解析】由题意知kx 2-6kx +(k +8)≥0在R 上恒成立.当k =0时,显然成立;当k >0时,Δ=(-6k )2-4k (k +8)≤0,得0<k ≤1.综上,实数k 的取值范围为[0,1].5. [-1,2] 【解析】因为y =f(x 2-1)的定义域为[-3,3],所以x ∈[-3,3],x 2-1∈[-1,2],所以y =f(x)的定义域为[-1,2].6. 15 【解析】因为A ⊆[8,16],所以8≤f(x)≤16对任意的x ∈[1,3]恒成立,所以⎩⎪⎨⎪⎧a ≤16x -x 2,a ≥8x -x 2对任意的x ∈[1,3]恒成立,当x ∈[1,3]时,16x -x 2∈[15,39],8x -x 2∈[7,15],所以⎩⎪⎨⎪⎧a ≤15,a ≥15,故a =15,即a 的值为15.7. 【解答】(1) 当x ∈⎣⎡⎦⎤12,2时,f(x)=a -1x ,所以函数f(x)在⎣⎡⎦⎤12,2上是增函数.所以f(x)的值域为⎣⎡⎦⎤a -2,a -12, 结合题设有⎩⎨⎧a -2=12,a -12=2,所以a =52.(2) 当x ∈[m ,n](m<n<0)时,f(x)=a +1x ,所以函数f(x)在[m ,n]上是减函数, 所以f(x)的值域为⎣⎡⎦⎤a +1n,a +1m , 假设存在实数a ,使得函数f(x)的定义域与值域均为[m ,n],则⎩⎨⎧a +1n=m ,a +1m =n.两式相减,得1m -1n =n -m ,即n -m mn=n -m ,因为m<n<0,所以mn =1,所以a =0.综上所述,存在实数a =0满足题设,此时mn =1. 8. 【解答】(1) f(x)=x +1x +3,x ∈[0,a](a>0). (2) 由(1)知函数f(x)的定义域为⎣⎡⎦⎤0,14. 令x +1=t ,则x =(t -1)2,t ∈⎣⎡⎦⎤1,32, 则f(x)=F(t)=tt 2-2t +4=1t +4t-2. 因为当t =4t 时,t =±2∉⎣⎡⎦⎤1,32. 又当t ∈⎣⎡⎦⎤1,32时,y =t +4t 单调递减, 故F(t)单调递增,所以F(t)∈⎣⎡⎦⎤13,613.所以函数f(x)的值域为⎣⎡⎦⎤13,613.第6课 函数的单调性A 应知应会1. ⎝⎛⎦⎤-∞,34 【解析】令u =2x 2-3x +1=2⎝⎛⎭⎫x -342-18. 因为u =2⎝⎛⎭⎫x -342-18在⎝⎛⎦⎤-∞,34上单调递减,函数y =⎝⎛⎭⎫13u在R 上单调递减,所以y =⎝⎛⎭⎫132x 2-3x +1在⎝⎛⎦⎤-∞,34上单调递增. 2. (3,+∞) 【解析】依题意得不等式f(x)<f(2x -3)等价于x <2x -3,解得x >3,即x 的取值范围是(3,+∞).3. 5 【解析】依题意可得函数图象的对称轴方程为x =a -12×2=1,所以a =5.4. ⎣⎡⎦⎤0,32 【解析】y =-(x -3)|x|=⎩⎪⎨⎪⎧-x 2+3x ,x>0,x 2-3x ,x ≤0.作出该函数的图象如图所示,观察图象可知函数的单调增区间为⎣⎡⎦⎤0,32.(第4题)5. ⎝⎛⎭⎫12,+∞ 【解析】设x 1>x 2>-2,则f(x 1)>f(x 2),又f(x 1)-f(x 2)=ax 1+1x 1+2-ax 2+1x 2+2=2ax 1+x 2-2ax 2-x 1(x 1+2)(x 2+2)=(x 1-x 2)(2a -1)(x 1+2)(x 2+2)>0.由x 1-x 2>0,x 1+2>0,x 2+2>0,知2a -1>0,所以a>12.6. 3 【解析】因为y =⎝⎛⎭⎫13x和y =-log 2(x +2)都是[-1,1]上的减函数,所以f(x)=⎝⎛⎭⎫13x-log 2(x +2)是在区间[-1,1]上的减函数,所以最大值为f(-1)=3.7. 【解答】设x 1,x 2是任意两个正数,且0<x 1<x 2,则f(x 1)-f(x 2)=⎝⎛⎭⎫x 1+a x 1-⎝⎛⎭⎫x 2+a x 2=x 1-x 2x 1x 2(x 1x 2-a). 当0<x 1<x 2≤a 时,0<x 1x 2<a ,又x 1-x 2<0,所以f(x 1)-f(x 2)>0,即f(x 1)>f(x 2), 所以函数f(x)在(0,a]上是减函数;当a ≤x 1<x 2时,x 1x 2>a ,又x 1-x 2<0, 所以f(x 1)-f(x 2)<0,即f(x 1)<f(x 2), 所以函数f(x)在[a ,+∞)上是增函数.综上可知,函数f(x)=x +ax (a >0)在(0,a]上是减函数,在[a ,+∞)上是增函数.8. 【解答】(1) 任取x 1<x 2<-2,则f(x 1)-f(x 2)=x 1x 1+2-x 2x 2+2=2(x 1-x 2)(x 1+2)(x 2+2).因为(x 1+2)(x 2+2)>0,x 1-x 2<0,所以f(x 1)<f(x 2), 所以f(x)在(-∞,-2)上单调递增. (2) 任取1<x 1<x 2,则f(x 1)-f(x 2)=x 1x 1-a -x 2x 2-a =a (x 2-x 1)(x 1-a )(x 2-a ).因为a>0,x 2-x 1>0,所以要使f(x 1)-f(x 2)>0,只需(x 1-a)(x 2-a)>0在(1,+∞)上恒成立,所以a ≤1. 综上所述,a 的取值范围是(0,1]. B 巩固提升 1. [3,+∞) 【解析】设t =x 2-2x -3,由t ≥0,即x 2-2x -3≥0,解得x ≤-1或x ≥3,所以函数f(x)的定义域为(-∞,-1]∪[3,+∞).因为函数t =x 2-2x -3的图象的对称轴为x =1,所以函数t =x 2-2x -3在(-∞,-1]上单调递减,在[3,+∞)上单调递增,所以函数f(x)的单调增区间为[3,+∞).2. (-∞,0)∪(1,+∞) 【解析】因为f(x)为R 上的减函数,且f ⎝⎛⎭⎫1x >f (1),所以1x <1.当x <0时,显然成立;当x >0时,得x >1,所以实数x 的取值范围是(-∞,0)∪(1,+∞).3. -6 【解析】由函数f(x)的图象可知,函数f(x)在(-∞,-a 2]上单调递减,在[-a 2,+∞)上单调递增,又函数f(x)的单调增区间是[3,+∞),所以-a2=3,解得a =-6.4. [0,1) 【解析】由题意知g(x)=⎩⎪⎨⎪⎧x 2,x>1,0,x =1,-x 2,x<1,其图象如图所示,由图象知单调减区间是[0,1).(第4题)5. [-2,0) 【解析】因为当x ≥1时,f(x)=-x 2+2ax -2a 是减函数,所以a ≤1.当x <1时,函数f(x)=ax +1是减函数,所以a <0,分界点处的值应满足-12+2a ×1-2a ≤1×a +1,解得a ≥-2,所以-2≤a <0.6. (-∞,0) 【解析】作出函数f(x)的图象如图所示,若f(x +1)<f(2x),则必有⎩⎪⎨⎪⎧2x<0,2x<x +1,解得x<0,所以满足f(x +1)<f(2x)的x 的取值范围是(-∞,0).(第6题)7. 【解答】(1) 由2f(1)=f(-1),得22-2a =2+a ,解得a =23. (2) 任取x 1,x 2∈[0,+∞),且x 1<x 2, f(x 1)-f(x 2)=x 21+1-ax 1-x 22+1+ax 2=x 21+1-x 22+1-a(x 1-x 2)=x 21-x 22x 21+1+x 22+1-a(x 1-x 2)=(x 1-x 2)⎝ ⎛⎭⎪⎫x 1+x 2x 21+1+x 22+1-a . 因为0≤x 1<x 21+1,0<x 2<x 22+1,所以0<x 1+x 2x 21+1+x 22+1<1.又因为a ≥1,所以f(x 1)-f(x 2)>0, 所以f(x)在[0,+∞)上单调递减.8. 【解答】(1) 当a =1时,f(x)=2x -1x,任取0<x 1<x 2≤1,则f(x 1)-f(x 2)=2(x 1-x 2)-⎝⎛⎭⎫1x 1-1x 2=(x 1-x 2)·⎝⎛⎭⎫2+1x 1x 2.因为0<x 1<x 2≤1,所以x 1-x 2<0,x 1x 2>0.所以f(x 1)<f(x 2),所以f(x)在(0,1]上单调递增,无最小值,当x =1时取得最大值1,所以f(x)的值域为(-∞,1].(2) 若a ≥0,y =f(x)在(0,1]上单调递增,无最小值,当x =1时取得最大值2-a.若a <0,f(x)=2x +-ax,当-a2≥1,即a ∈(-∞,-2]时,y =f(x)在(0,1]上单调递减,无最大值,当x =1时取得最小值2-a ;当-a2<1,即a ∈(-2,0)时,y =f(x)在⎝⎛⎭⎫0,-a 2上单调递减,在⎣⎡⎦⎤-a 2,1上单调递增,无最大值,当x =-a2时取得最小值2-2a.第7课 函数的奇偶性A 应知应会1. 2 【解析】因为偶函数的定义域应当关于原点对称,故t -4=-t ,解得t =2. 2. 12 【解析】因为f(x)为奇函数,所以f(-x)=-f(x),即12-x -1+a =-12x -1-a ,化简得2a =1,解得a =12.3. 奇函数 【解析】显然f(x)的定义域为(-1,1),关于原点对称.又因为f(-x)=ln (1-x)-ln (1+x)=-f(x),所以f(x)为奇函数.4. 27 【解析】由f(-7)=-17,得g(-7)=-22,根据g(x)为奇函数,得g(7)=22,又f(7)=g(7)+5,所以f(7)=22+5=27.5. -2 【解析】因为函数f(x)是定义在R 上的奇函数,所以f (0)=0.又因为当x <0时,f (x )=log 2(2-x ),所以f (2)=-f (-2)=-log 2(2+2)=-2,故f (0)+f (2)=-2.6. (-∞,2] 【解析】由f(x)在R 上是奇函数且在(-∞,0]上单调递增,知f (x )在R 上单调递增.又f (-1)=-2,则f (1)=2,所以f (2x -3)≤2=f (1),所以2x -3≤1,即x ≤2.7. 【解答】因为f(x)是定义在R 上的奇函数,可得f (0)=-f (0),所以f (0)=0.当x >0时,-x <0,由已知得f (-x )=x lg(2+x ), 所以-f (x )=x lg(2+x ),即f (x )=-x lg(2+x )(x >0).所以f (x )=⎩⎪⎨⎪⎧-x lg (2-x ),x <0,-x lg (2+x ),x ≥0.即f (x )=-x lg(2+|x |)(x ∈R ).8. 【解答】(1) 当a =0时,f(x)=x 2,对任意x ∈(-∞,0)∪(0,+∞),有f(-x)=(-x)2=x 2=f(x),所以f(x)为偶函数. 当a ≠0时,f(x)=x 2+ax,f(-1)=1-a ,f(1)=1+a ,所以f(-1)≠-f(1),f(-1)≠f(1),所以函数f(x)既不是奇函数也不是偶函数.综上所述,当a =0时,f(x)为偶函数;当a ≠0时,f(x)为非奇非偶函数.(2) 设2≤x 1<x 2,则f(x 1)-f(x 2)=x 21+a x 1-x 22-a x 2=x 1-x 2x 1x 2[x 1x 2(x 1+x 2)-a],要使函数f(x)在x ∈[2,+∞)上为增函数,则f(x 1)-f(x 2)<0恒成立.因为x 1-x 2<0,x 1x 2>4, 所以a<x 1x 2(x 1+x 2)恒成立.又因为x 1+x 2>4,所以x 1x 2(x 1+x 2)>16, 所以实数a 的取值范围是(-∞,16].B 巩固提升1. 1 【解析】由题知y =ln (x +a +x 2)是奇函数,所以ln (x +a +x 2)+ln (-x +a +x 2)=ln (a +x 2-x 2)=ln a =0,解得a =1.2. -3 【解析】因为f(x)为定义在R 上的奇函数,所以f (0)=0,即f (0)=20+m =0,解得m =-1,则f (-2)=-f (2)=-(22-1)=-3.3. [1,3] 【解析】因为f(x)为奇函数,所以f(-1)=1,不等式-1≤f(x -2)≤1,即f(1)≤f(x -2)≤f(-1),因为f(x)在R 上单调递减,所以-1≤x -2≤1,解得1≤x ≤3,故x 的取值范围为[1,3].4. [-1,3] 【解析】易知偶函数f(x)在[0,+∞)上单调递增,且f(2)=2,所以f(x -1)≤2=f(2),即|x -1|≤2,所以-1≤x ≤3.5. (-5,0)∪(5,+∞) 【解析】由于f(x)为R 上的奇函数,所以当x =0时,f (0)=0;当x <0时,-x >0,所以f (-x )=x 2+4x =-f (x ),即f (x )=-x 2-4x ,所以f (x )=⎩⎪⎨⎪⎧x 2-4x ,x >0,0,x =0,-x 2-4x ,x <0.由f (x )>x ,可得⎩⎪⎨⎪⎧x 2-4x >x ,x >0或⎩⎪⎨⎪⎧-x 2-4x >x ,x <0,解得x >5或-5<x <0,所以原不等式的解集为(-5,0)∪(5,+∞).6. ④ 【解析】依题意知性质(1)反映函数f(x)在定义域上为奇函数,性质(2)反映函数f(x)在定义域上为减函数.①f(x)=1x 为定义域上的奇函数,但不是定义域上的减函数,其单调减区间为(-∞,0),(0,+∞),排除①;②f(x)=x 2为定义域上的偶函数,排除②;③f(x)=2x -12x+1=1-22x +1的定义域为R ,由于y =2x +1在R 上为增函数,故函数f (x )为R 上的增函数,排除③;④根据f (x )=⎩⎪⎨⎪⎧-x 2,x ≥0,x 2,x <0的图象,显然此函数为奇函数,且在定义域上为减函数,故④为“理想函数”.7. 【解答】(1) 显然f(x)的定义域是R ,关于原点对称.在f (x +y )=f (x )+f (y )中,令y =-x ,得f (0)=f (x )+f (-x ).令x =y =0,得f (0)=f (0)+f (0),所以f (0)=0,所以f (x )+f (-x )=0,即f (-x )=-f (x ),所以f (x )是奇函数.(2) 由f (-3)=a ,f (x +y )=f (x )+f (y ),及f (x )是奇函数,得f (12)=2f (6)=4f (3)=-4f (-3)=-4a .8. 【解答】(1) 由题意得-3x +13x +1+1=3x,化简得3·(3x )2+2·3x -1=0,解得3x =-1(舍去)或3x =13,从而x =-1.(2) 因为f(x)是奇函数,所以f(-x)+f(x)=0, 所以-3-x +a 3-x +1+b +-3x +a 3x +1+b=0,化简并变形得(3a -b)(3x +3-x )+2ab -6=0.要使上式对任意的x 恒成立,则3a -b =0且2ab -6=0,解得⎩⎪⎨⎪⎧a =1,b =3或⎩⎪⎨⎪⎧a =-1,b =-3,因为f(x)的定义域是R ,所以⎩⎪⎨⎪⎧a =-1,b =-3不合题意,所以a =1,b =3,所以f (x )=-3x +13x +1+3=13⎝⎛⎭⎫-1+23x +1,对任意x 1,x 2∈R ,x 1<x 2,有f (x 1)-f (x 2)=13⎝⎛⎭⎫23x 1+1-23x 2+1=23·3x 2-3x 1(3x 1+1)(3x 2+1). 因为x 1<x 2,所以3x 2-3x 1>0,所以f (x 1)>f (x 2),因此f (x )在R 上单调递减.因为f (t 2-2t )<f (2t 2-k ),所以t 2-2t >2t 2-k , 即t 2+2t -k <0在R 上有解, 所以Δ=4+4k >0,解得k >-1. 所以k 的取值范围为(-1,+∞).第8课 函数的图象和周期性A 应知应会 1. 2.5 【解析】由f(x +2)=-f(x),得f(x +4)=f((x +2)+2)=-f(x +2)=-[-f(x)]=f(x),所以函数f(x)的周期为4,所以f(105.5)=f(4×27-2.5)=f(-2.5)=f(2.5)=2.5.2. y =(x -1)2+3 【解析】把函数y =f(x)的图象向左平移1个单位长度,即把其中x 换成x +1,于是得y =[(x +1)-2]2+2=(x -1)2+2的图象,再向上平移1个单位长度,即得到y =(x -1)2+2+1=(x -1)2+3的图象.3. 116 【解析】f(2)=f(3)=f(4)=⎝⎛⎭⎫124=116.4. (-∞,0]∪(1,2] 【解析】y =f(x +1)的图象向右平移1个单位长度得到y =f(x)的图象,由已知可得f(x)的图象的对称轴方程为x =1,过定点(2,0),且函数在(-∞,1)上单调递减,在(1,+∞)上单调递增,则f(x)的大致图象如图所示.不等式(x -1)f(x)≤0可化为⎩⎪⎨⎪⎧x>1,f (x )≤0或⎩⎪⎨⎪⎧x<1,f (x )≥0.由图可知符合条件的解集为(-∞,0]∪(1,2].(第4题)5. 1 【解析】f(x +2)=f(x)⇒T =2,由f ⎝⎛⎭⎫-52-f ⎝⎛⎭⎫92=0,得f ⎝⎛⎭⎫-12=f ⎝⎛⎭⎫12,4-12+a =14-log 212⇒a =34,因此f(4a)=f(3)=f(-1)=4-1+34=1.6. 【解答】(1) y =2x -1x -1=2(x -1)+1x -1=2+1x -1. 先作出函数y =1x 的图象,再把函数y =1x 的图象向右平移1个单位长度后得到函数y =1x -1的图象,最后把函数y =1x -1的图象向上平移2个单位长度后得到函数y =2+1x -1的图象,如图(1)所示.(2) y =(x +1)|x -2|=⎩⎪⎨⎪⎧-x 2+x +2,x<2,x 2-x -2,x ≥2.函数的图象如图(2)所示.(3) 首先作出函数y =2x 的图象,在y 轴右边的保持不变,去掉y 轴左边的图象,再把y轴右边的图象对称地翻折到y 轴左边,即得函数y =2|x|的图象,最后把函数y =2|x|的图象向左平移1个单位长度后得到函数y =2|x +1|的图象,如图(3)所示.图(2)图(3)(第6题)7. 【解答】(1) 由函数f(x)的图象关于直线x =1对称,知f(x +1)=f(1-x),即有f(-x)=f(x +2).又函数f(x)是定义在R 上的奇函数,则f (-x )=-f (x ), 故f (x +2)=-f (x ),从而f (x +4)=-f (x +2)=f (x ), 即函数f (x )是以4为周期的周期函数.(2) 由函数f (x )是定义在R 上的奇函数,得f (0)=0.当x ∈[-1,0)时,-x ∈(0,1],f (x )=-f (-x )=--x . 故当x ∈[-1,0]时,f (x )=--x . 当x ∈[-5,-4]时,x +4∈[-1,0],f (x )=f (x +4)=--x -4.从而当x ∈[-5,-4]时,函数f (x )=--x -4. B 巩固提升1. 4 【解析】由f(x)·f(x +2)=13,得f(x +2)=13f (x ),所以f(x +4)=f((x +2)+2)=13f (x +2)=f(x),所以f(x)是以4为周期的周期函数.2. 1 【解析】由题意可知f ⎝⎛⎭⎫32=f ⎝⎛⎭⎫2-12=f ⎝⎛⎭⎫-12=-4×⎝⎛⎭⎫-122+2=1. 3. (-1,1] 【解析】如图,作出函数y =log 2(x +1)的图象,当x =1时,两图象相交,由图象知不等式的解集为{x|-1<x ≤1}.(第3题)4. g(x)=3x -2 【解析】设g(x)上的任意一点A(x ,y),则该点关于直线x =1的对称点为B(2-x ,y),而该点在f(x)的图象上.所以y =⎝⎛⎭⎫132-x=3x -2,即g(x)=3x -2.5. [0,2) 【解析】方法一:由于平移不改变值域,故只需要研究原函数的值域.画出函数f(x)=|2x -2|的图象如图所示,由图易得值域为[0,2).方法二:因为x ∈(-1,2),所以2x ∈⎝⎛⎭⎫12,4,2x -2∈⎝⎛⎭⎫-32,2,所以|2x -2|∈[0,2).因为y =f(x -1)是由f(x)向右平移1个单位长度得到的,所以值域不变,所以y =f(x -1)的值域为[0,2).(第5题)6. 1 516 【解析】因为函数y =f(x)是最小正周期为4的偶函数,且在x ∈[-2,0]时,f(x)=2x +1,所以函数的值域为[-3,1],对任意x i ,x j (i ,j =1,2,3,…,n),都有|f(x i )-f(x j )|≤f(x)max -f(x)min =4,要使n +x n 取得最小值,尽可能多让x i (i =1,2,3,…,n)取得最值,且f(0)=1,f(2)=-3,因为0≤x 1<x 2<…<x n ,|f(x 1)-f(x 2)|+|f(x 2)-f(x 3)|+…+|f(x n-1)-f(x n )|=2 020,所以n 的最小值为2 0204+1=506,相应的x n 最小值为1 010,则n +x n 的最小值为1 516.7. 【解答】(1) 因为f(x +2)=-f(x),所以f(x +4)=-f(x +2)=f(x). 所以f(x)的最小正周期为4.(2) f(0)=0,f(1)=1,f(2)=0,f(3)=f(-1)=-f(1)=-1. 又因为f(x)是周期为4的周期函数,所以f(0)+f(1)+f(2)+f(3)=f(4)+f(5)+f(6)+f(7)=…=f(2 012)+f(2 013)+f(2 014)+f(2 015)=0,所以f(0)+f(1)+f(2)+…+f(2 018)=f(2 016)+f(2 017)+f(2 018)=f(0)+f(1)+f(2)=1.8. 【解答】 (1) 因为f(4)=0,所以4|m -4|=0,即m =4.(2) 因为f(x)=x|4-x|=⎩⎪⎨⎪⎧x 2-4x ,x ≥4,-x 2+4x ,x<4.即f(x)=⎩⎪⎨⎪⎧(x -2)2-4,x ≥4,-(x -2)2+4,x<4, 所以函数f(x)的图象如图所示.由图象知函数f(x)有两个零点.(3) 从图象上观察可知,f(x)的单调减区间为[2,4].(4) 从图象上观察可知,不等式f(x)>0的解集为{x|0<x<4或x>4}.(5) 由图象可知若y =f(x)与y =m 的图象有三个不同的交点,则0<m<4,所以集合M ={m|0<m<4}.(第8题)第9课 二次函数、幂函数A 应知应会1. 13 【解析】设幂函数为f(x)=x α,由图象经过点⎝⎛⎭⎫-2,-18,得-18=(-2)α,所以α=-3,所以f(x)=x-3,令x -3=27,得x =13.2. [-6,12] 【解析】y =2(x -2)2-6,当x =2时,y 取得最小值,为-6;当x =-1时,y 取得最大值,为12.3. 2 【解析】由题意知m 2-m -1=1,解得m =2或m =-1.当m =2时,m 2-2m -3=-3,f(x)=x -3,符合题意;当m =-1时,m 2-2m -3=0,f(x)=x 0,不合题意.综上,m =2.4. {x|-4≤x ≤4} 【解析】由f ⎝⎛⎭⎫12=22⇒α=12,故f(|x|)≤2⇒|x|12≤2⇒|x|≤4,故其解集为{x|-4≤x ≤4}.5. [7,+∞) 【解析】易知函数f(x)的图象开口向上,对称轴方程为x =a -12,因为函数f(x)在区间⎝⎛⎭⎫12,1上为增函数,所以a -12≤12,解得a ≤2,所以f(2)=4-(a -1)×2+5≥7,即f(2)≥7.6. 7 【解析】因为f(0)=4,所以a +2b =4,即a =4-2b ,所以f(1)=ab +a +2b +1=ab +5=(4-2b)b +5=-2b 2+4b +5=-2(b -1)2+7,所以当b =1时,f(1)的最大值为7.7. 【解答】作出函数y =x 2-2x +3的图象如图所示.由图象可知,要使函数在[0,m]上取得最小值2,则1∈[0,m],从而m ≥1,当x =0时,y =3;当x =2时,y =3, 所以要使函数的最大值为3,则m ≤2, 故实数m 的取值范围为[1,2].(第7题)8. 【解答】(1) 由题意知f(-1)=a -b +1=0,且-b2a =-1,所以a =1,b =2,所以f(x)=x 2+2x +1,其单调减区间为(-∞,-1],单调增区间为[-1,+∞).(2) f(x)>x +k 在区间[-3,-1]上恒成立, 即x 2+x +1>k 在[-3,-1]上恒成立.设g(x)=x 2+x +1,x ∈[-3,-1],有k <g(x)min . 因为g(x)在[-3,-1]上单调递减, 所以g(x)min =g(-1)=1.所以k <1,即k 的取值范围为(-∞,1). B 巩固提升1. ⎩⎨⎧⎭⎬⎫a ⎪⎪a<-1或23<a<32 【解析】因为函数f(x)在(0,+∞)上单调递减,所以m 2-2m -3<0,解得-1<m<3.因为m ∈N *,所以m =1,2.又函数f (x )的图象关于y 轴对称,所以m 2-2m -3是偶数,而22-2×2-3=-3为奇数,12-2×1-3=-4为偶数,所以m =1.因为f (x )=x -13在(-∞,0),(0,+∞)上均为减函数,所以(a +1)-13<(3-2a )-13等价于a +1>3-2a >0或0>a +1>3-2a 或a +1<0<3-2a ,解得a <-1或23<a <32.故a 的取值范围为⎩⎨⎧⎭⎬⎫a ⎪⎪a <-1或23<a <32. 2. (-4,0] 【解析】当m =0时,显然成立;当m ≠0时,⎩⎪⎨⎪⎧m <0,Δ=(-m )2+4m <0,解得-4<m <0.综上可知,实数m 的取值范围是(-4,0].3. 9 【解析】由于f(x 1)=f(x 2),所以二次函数f(x)的对称轴为x =x 1+x 22=-b2a ,所以f(x 1+x 2)=f ⎝⎛⎭⎫-ba =9. 4. f(x)=-3x 2+12x 【解析】方法一:设f(x)=ax 2+bx +c(a ≠0),由f(x)>0的解集是(0,4),可知f(0)=f(4)=0,且二次函数的图象开口向下,对称轴方程为x =2,再由f(x)在区间[-1,5]上的最大值是12,可知f(2)=12,即⎩⎪⎨⎪⎧f (0)=0,f (4)=0,f (2)=12,解得⎩⎪⎨⎪⎧a =-3,b =12,c =0.所以f(x)=-3x 2+12x.方法二:由f(x)>0的解集是(0,4),可设f(x)=ax(x -4)(a<0),f(x)在区间[-1,5]上的最大值为f(2)=12,即-4a =12,所以a =-3,所以f(x)=-3x 2+12x.5. [1,1+2] 【解析】f(x)=x|x -2|=⎩⎪⎨⎪⎧x 2-2x ,x>2,-x 2+2x ,x ≤2,作出f(x)的图象如图所示,当x>2时,令f(x)=1,得x =1+ 2.因为x ∈[0,a]时,值域为[0,1],所以1≤a ≤1+ 2.(第5题)6. (-1,3) 【解析】根据题意,f(x)是定义在R 上的奇函数,则有f (0)=0,当x <0时,f (x )=x 2-2x +1=(x -1)2为减函数,则当x >0时,f (x )也为减函数,综上可得f (x )在R 上为减函数.若f (x 2-3)>f (2x ),则有x 2-3<2x ,解得-1<x <3,即不等式f (x 2-3)>f (2x )的解集为(-1,3).7. 【解答】若a =0,则f(x)=-2x 在[0,1]上单调递减,所以f(x)min =f(1)=-2. 若a ≠0,f(x)=a ⎝⎛⎭⎫x -1a 2-1a. 当a >0时,函数f(x)的图象的开口方向向上,且对称轴为x =1a .当1a ≤1,即a ≥1时,函数f(x)的图象的对称轴在[0,1]内,所以f(x)在⎣⎡⎦⎤0,1a 上单调递减,在⎣⎡⎦⎤1a ,1上单调递增, 所以f(x)min =f ⎝⎛⎭⎫1a =-1a. 当1a >1,即0<a <1时,函数f(x)的图象的对称轴在[0,1]的右侧,所以f(x)在[0,1]上单调递减,所以f(x)min =f(1)=a -2.当a <0时,函数f(x)的图象的开口方向向下,且对称轴x =1a <0,在y 轴的左侧,所以f(x)在[0,1]上单调递减,所以f(x)min =f(1)=a -2.综上所述,f(x)min =⎩⎪⎨⎪⎧a -2,a <1,-1a,a ≥1.8. 【解答 】(1) 由题意得x 1+x 2=-1a ,x 1x 2=1a ,所以(1+x 1)·(1+x 2)=1+(x 1+x 2)+x 1x 2=1-1a +1a=1.(2) 令f(x)=ax 2+x +1(a >0), 由Δ=1-4a ≥0,得0<2a ≤12,所以函数f(x)图象的对称轴方程为x =-12a≤-2<-1.又f(-1)=a>0,所以f(x)的图象与x 轴的交点都在点(-1,0)的左侧,故x 1<-1且x 2<-1.(3) 由(1)知x 1=11+x 2-1=-x 21+x 2,所以x 1x 2=-11+x 2∈⎣⎡⎦⎤110,10,所以-1x 2∈⎣⎡⎦⎤111,1011, 所以a =1x 1x 2=-1+x 2x 2·1x 2=-1+x 2x 22=-⎣⎡⎦⎤⎝⎛⎭⎫-1x 2-122+14,故当-1x 2=12时,a 取得最大值14. 第10课 指数与指数函数A 应知应会1. (-∞,1) 【解析】原不等式等价于23-2x <24-3x ,所以3-2x<4-3x ,解得x<1. 2. c>a>b 【解析】因为函数y =0.6x 是减函数,且0<0.6<1.5,所以1=0.60>0.60.6>0.61.5,即b<a<1.因为函数y =1.5x 在(0,+∞)上是增函数,0.6>0,所以1.50.6>1.50=1,即c>1.综上,c>a>b.3. ⎣⎡⎭⎫12,+∞ 【解析】因为g(x)=2x -x 2=-(x -1)2+1≤1,所以函数y =⎝⎛⎭⎫122x -x 2的值域为⎣⎡⎭⎫12,+∞. 4. (-2,0) 【解析】方法一:因为函数y =a x (a>0,a ≠1)的图象恒过点(0,1),将该图象向左平移2个单位长度,再向下平移1个单位长度得到y =a x +2-1(a>0,a ≠1)的图象,所以y =a x +2-1(a>0,a ≠1)的图象恒过点(-2,0).方法二:令x +2=0,得x =-2,f(-2)=a 0-1=0,所以y =a x +2-1(a>0,a ≠1)的图象恒过点(-2,0).5. 15 【解析】由102x =25⇒(10x )2=25⇒10x =5⇒10-x =15. 6. (-1,2) 【解析】原不等式变形为m 2-m <⎝⎛⎭⎫12x,因为函数y =⎝⎛⎭⎫12x在(-∞,-1]上是减函数,所以⎝⎛⎭⎫12x ≥⎝⎛⎭⎫12-1=2,当x ∈(-∞,-1]时,m 2-m <⎝⎛⎭⎫12x恒成立等价于m 2-m <2,解得-1<m <2.7. 【解答】(1) 原式=(0.1)4×⎝⎛⎭⎫-14+33×23-⎝⎛⎭⎫132×⎝⎛⎭⎫-32-1=(0.1)-1+32-27-1=-9.(2) 原式=(a 3b 2a 13b 23)12ab 2a -13b 13=a 32+16-1+13·b1+13-2-13=ab -1. 8. 【解答】(1) 因为f(x)=b·a x 的图象过点A(1,6),B(3,24),所以⎩⎪⎨⎪⎧b·a =6,①b·a 3=24,②②÷①得a 2=4.又a>0且a ≠1,所以a =2,b =3, 所以f(x)=3·2x .(2) 由(1) 知⎝⎛⎭⎫1a x +⎝⎛⎭⎫1b x -m ≥0在(-∞,1]上恒成立可转化为m ≤⎝⎛⎭⎫12x +⎝⎛⎭⎫13x在(-∞,1]上恒成立.令g(x)=⎝⎛⎭⎫12x+⎝⎛⎭⎫13x,则g(x)在(-∞,1]上单调递减, 所以m ≤g(x)min =g(1)=12+13=56,故实数m 的取值范围是⎝⎛⎦⎤-∞,56. B 巩固提升1. 100 【解析】原式=⎝⎛⎭⎫25912+10.12+⎝⎛⎭⎫6427-23-3+3748=53+100+916-3+3748=100.2. a ≤b 【解析】由题意得f(a)≥2a .因为f(a)≤2b ,则2a ≤f(a)≤2b ,所以2a ≤2b .又y =2x是R 上的增函数,所以a ≤b .3. ⎝⎛⎭⎫-1,-12 【解析】y =a ⎝⎛⎭⎫2x -12-2x ,令2x -12=0,则y +2x =0,得x =-1,y =-12,所以这个定点的坐标为⎝⎛⎭⎫-1,-12. 4. (log 23,+∞) 【解析】由题意知,当2a >a 2-a 2+3,即a>log 23时,存在x 1,x 2∈R ,使得f (x 1)=f (x 2).(第5题)5. 2 【解析】设2 017a =2 018b =t ,如图所示,由函数图象可得若t>1,则有a>b>0;若t =1,则有a =b =0;若0<t<1,则有a<b<0.故①②⑤可能成立,而③④不可能成立.6. e 【解析】由于f(x)=max {e |x|,e |x -2|}=⎩⎪⎨⎪⎧e x ,x ≥1,e 2-x ,x<1.当x ≥1时,f(x)≥e ,且当x =1时,取得最小值e ;当x<1时,f(x)>e ,故f(x)的最小值为f(1)=e .7. 【解答】(1) 因为f(x)是定义在R 上的奇函数,所以f (0)=0,即-1+b2+a =0,解得b =1,所以f (x )=-2x +12x +1+a.又由f (1)=-f (-1),知-2+14+a =--12+11+a ,解得a =2.经检验,当a =2,b =1时,f (x )为奇函数. (2) 由(1)知f (x )=-2x +12x +1+2=-12+12x +1,易知f (x )在(-∞,+∞)上为减函数.又因为f (x )是奇函数,所以不等式f (t 2-2t )+f (2t 2-1)<0等价于f (t 2-2t )<-f (2t 2-1)=f (-2t 2+1),从而t 2-2t >-2t 2+1,即3t 2-2t -1>0,解得t >1或t <-13.故不等式的解集为⎝⎛⎭⎫-∞,-13∪(1,+∞). 8. 【解答】(1) 函数f(x)=3x +λ·3-x的定义域为R .因为f (x )为奇函数,所以f (-x )+f (x )=0对任意的x ∈R 恒成立,即3-x +λ·3x +3x +λ·3-x =(λ+1)(3x +3-x )=0对任意的x ∈R 恒成立,所以λ=-1.由f (x )=3x -3-x >1,得(3x )2-3x -1>0, 解得3x >1+52或3x <1-52(舍去),所以不等式f (x )>1的解集为⎩⎨⎧⎭⎬⎫x ⎪⎪⎪x >log 31+52. (2) 由f (x )≤6,得3x +λ·3-x ≤6,即3x +λ3x ≤6.令t =3x ∈[1,9],则问题等价于t +λt ≤6对t ∈[1,9]恒成立,即λ≤-t 2+6t 对t ∈[1,9]恒成立. 令g (t )=-t 2+6t ,t ∈[1,9],因为g (t )在[1,3]上单调递增,在[3,9]上单调递减, 所以当t =9时,g (t )有最小值g (9)=-27,所以λ≤-27,即实数λ的取值范围为(-∞,-27].第11课 对数的运算A 应知应会1. 12 【解析】log 22=log 2212=12log 22=12. 2. 2 【解析】2log 510+log 50.25=log 5100+log 50.25=log 525=2. 3. lg 3 【解析】令3=10x ,则x =lg 3.4. a -2 【解析】log 38-2log 36=log 323-2(log 32+log 33)=3log 32-2(log 32+1)=log 32-2=a -2.5. 2 【解析】原方程等价于⎩⎪⎨⎪⎧x>0,x +3>0,x (x +3)=10,解得x =2.6. b -a +1a 【解析】log 215=lg 15lg 2=lg ⎝⎛⎭⎫32×10lg 2=lg 3-lg 2+1lg 2=b -a +1a.7. 【解答】(1) 原式=log 535+log 550-log 514+2log 12212。
南方凤凰台一轮复习资料

南方凤凰台一轮复习资料南方凤凰台是国内较为知名的公共电视台之一,一直致力于为广大观众提供高质量的新闻、教育、文化、娱乐等节目。
其中,南方凤凰台也开设了一些备考、复习的相关教育节目,尤其是备考资料的方面做得非常出色。
南方凤凰台的备考资料主要包括视频课程、在线答疑、模拟考试等多种形式,覆盖了高中、大学等不同年级及各类考试。
其中比较具有代表性的包括高考、研究生入学考试、英语四、六级考试、司法考试、医学考试等。
南方凤凰台的备考资料有以下几个优势。
首先,南方凤凰台的备考资料覆盖面广、深度深。
不同于其他备考机构或者网站只提供零散的一些知识点或者试题,南方凤凰台的备考资料涵盖了整个考试课程的教学内容,同时将不同知识点之间的关联性进行了深入讲解,有助于学生更好地理解和掌握知识。
其次,南方凤凰台的备考资料采用的授课形式独特而生动,具有很强的趣味性。
其教师不仅有着丰富的授课经验,而且个个都是一线的名师,其中不乏一些国内知名的名师。
同时,南方凤凰台教师在授课过程中的生动幽默,不仅可以调动学生的学习兴趣,而且有助于提升学生的学习效率。
第三,南方凤凰台的备考资料在教学内容和试题形式上注重实用性和针对性。
教学内容不仅囊括了各种考试的基础课程内容,而且还会结合最新出的考试信息,及时调整教学内容和课程设置。
同时,南方凤凰台的备考试题不仅有着高度的代表性,而且试题类型和难度也很贴近实际考试,有助于学生充分了解考试题型和考试难度,进而掌握备考策略。
第四,南方凤凰台的备考资料可以随时随地进行学习。
南方凤凰台提供在线观看视频课程的服务,而且学习时间和地点也非常灵活,不受时间和空间限制。
此外,南方凤凰台还提供在线答疑系统,有助于学生及时解决自己的疑难问题,加强对知识的理解和掌握。
总之,南方凤凰台的备考资料具有非常高的实用性和科学性,不仅可以为考生的备考提供很好的帮助,而且也有助于提升学生的学习兴趣和学习动力。
希望广大考生能够充分利用南方凤凰台的教育资源,冲刺理想的考试成绩。
【南方新课堂 备战】高考英语一轮复习 综合能力检测(含详解) 新人教必修4

必修四综合能力检测(时间:120分钟满分:135分得分:________)Ⅰ语言知识及应用(共两节,满分45分)第一节完形填空(共15小题;每小题2分,满分30分)阅读下面短文,掌握其大意,然后从1-15各题所给的A、B、C和D项中,选出最佳选项。
“When a customer enters my store, forget me.He is King,” said John Wanamaker.This revolutionary concept __1__ the face of retailing (零售业) and led to the development of advertising and marketing as we know it today.But convincing as that slogan was, in truth the shopper was cheated out of the crown.Although manufacturing efficiency increased the __2__ of goods and lowered prices, people still relied on advertisements to get most __3__ about products.Through much of the past century, ads spoke to an audience restricted to just a few radio or television channels or a __4__ number of publications.Now media choice has __5__ too, and consumers select what they want from a __6__ greater variety of sources—especially with a few__7__ of a computer mouse.Thanks to the Internet, the consumer is finally __8__ power.As our survey shows, customer __9__ has great implications for companies, because it is changing the way the world shops.Many firms already claim to be “customer__10__” or “consumercentered”.Now their __11__ will be tested as never before.Taking advantage of shoppers' __12__ will no longer be possible: people will tell others, even those without the Internet, that prices in the next town are __13__ or that certain goods are inferior.The Internet is working wonders in__14__ standards.Good and __15__ firms should benefit most.1.A.changed B.maintainedC.restored D.rescued2.A.quality B.varietyC.weight D.price3.A.bargain B.certificateC.change D.information4.A.limited B.minimumC.sufficient D.great5.A.disappeared B.existedC.exploded D.survived6.A.quite B.littleC.far D.very7.A.clicks B.typistsC.changes D.designs8.A.losing B.catchingC.controlling D.seizing9.A.power B.qualityC.package D.quantity10.A.driven B.criticizedC.helped D.chased11.rmation B.investmentC.claims D.shops12.A.generosity B.knowledgeC.curiosity D.ignorance13.A.higher B.unreasonableC.unfair D.cheaper14.A.raising B.loweringC.abandoning D.carrying15.A.nice B.honestC.new D.old第二节语法填空(共10小题;每小题1.5分,满分15分)阅读下面短文,按照句子结构的语法性和上下文连贯的要求,在空格处填入一个适当的词或使用括号中词语的正确形式填空。
2024届高考英语一轮复习选择性必修第一册UNIT5WORKING THE LAND课时作业含答案

UNIT5WORKING THE LANDⅠ.阅读理解A(湖南省三湘名校教育联盟2022届高三第一次大联考)Teaching the next generation is an effective way to change the future. A nonprofit organization in Israel has taken this to heart by greening schools with soilless farming. It is called Startup Roots, which is teaching students about hydroponics(水培), and as a result, they are learning nutrition and science.“Many children think that vegetables come from the grocery store. They have no concept of the chain behind it. So we try to connect kids with the source of their food,”said Robin Katz, founder of this organization. Katz started it in 2014,realizing that many children have no access to fresh, healthy and affordable produce. She was drawn to hydroponics because this system uses 90 percent less water and grows more plants per meter than traditional farming.Their first project created a soilless farm using just 100 square meters of a school. They started out by growing 14 plants per meter. The students were excited to see results in just 30 days and were soon able to produce 1,500 vegetables per month!Working with science teachers, the organization guides students to learn science and nutrition. In addition, it has brought in nutritionists to teach the effect that food choices have on health. It soon improved their methods by fixing vertical farming systems. Since then, their production has improved greatly, resulting in the production of 120 plants per meter.In order to educate the general population, the organization is also working to create an interactive urban agriculture exhibit, which is transforming students by turning them into interactive learners. More youth have become so fascinated by the process, they are eagerly researching plant seeds and are growing plants.“There is no greater pleasure than to watch students grow by growing,” said Katz. Aside from producing organic vegetables in schools, the organization is planting roots in this young generation, inspiring them to lead healthier and more environmentally-friendly lives.1.Why did Robin Katz show interest in hydroponics?A.It took less land than traditional farming.B.It caused no damage to the environment.C.It was easy to produce good vegetables.D.It saved water and had a higher yield.2.What can we learn about the first soilless farm?A.It produced 120 plants per meter.B.It relied on vertical farming systems.C.It produced vegetables in a month.D.It was designed by science teachers.3.What is the final influence of the new farming system?A.Students will grow better plants.B.Students will change their lifestyle.C.Students will protect environment.D.Students will eat organic vegetables.4.Which of the following is a suitable title for the text?A.Inspiring Future Hydroponic FarmingB.A Soilless Farm on ExhibitionC.Researching Plant Seeds in FutureD.Helping Students Grow Better【语篇解读】本文是一篇说明文,讲述了以色列一家非营利组织通过新耕作制度(水培法),让学生了解营养和科学,并鼓励学生过更健康环保的生活。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
31.B句意:经常实现你开始要做的事并不重要。set out to do sth:(为达到某个结果而)开始做某事。look forward to和set about后都接名词或动名词(短语);没有give in to do sth的用法。
32. B根据文章第四段中的“We plan to dig a hole all the way through the earth!”可知,他们想挖一个穿过 1
5. make good use of 6 informed of
7. to approve of 8. was charged with
9. graduated from 10. look back on
二、11. next to; whose 12. less; than
13. took me; to read 14. During, e-mail; for free
29.D upon+n. /doing:一??就??,相当于一个由as soon引导的时间状语从甸。A项壮soon as后应接从句;C项后应加at;而B项中的介词upon后应该接动名词,
30.B该句为典型的动名词作主语并且用It作形式主语,将真正的主语(动名词短语)后移的句子。
五、 实现既定目标固然重要,但对于大多数人来说,朝着自己的目标而努力的过程也一样重要。因为在这一过程中你会发现很多有价值的东西,它们也套让你的生命变得重有意义。
15. give up; that
三、 17.I ntil it was too late
四、根据句意可知第一空是“有经验的老师”式。
22.A ma
way。
23.D考查动词词义辨析。:取得,获得;finish:结束;conclude,得出结论。
24C
25.A
26.B7——请自便。if you please常用于客气的请求t意为“如果你愿意”;:。。re:非常乐意,表示愉快地接受别人让自己做的事;it's my pleasure:27穿着奇怪衣服的过路人身上。draw sb’s :句子中who引导的定语从句含有had和were两个并列的谓语动词,28.Aneeded的宾语,因此用what.
HCO内部资料,严禁外泄
江苏高考总复习一轮配套检测与评估
英语详解详析
Module 1
Unit 1 School life
一、1. show respect for 2. was devoted to
3. earn my living 4. was donated to
