加拿大国际袋鼠数学竞赛试题 -2004年
2024袋鼠数学b级考试真题

2024袋鼠数学b级考试真题今天咱们来聊聊2024年袋鼠数学B级考试真题。
这对咱们来说可是个很有趣的话题呢。
你们知道吗,袋鼠数学竞赛就像是一场充满趣味的数学大冒险。
B级的考试真题里有好多特别的题目。
比如说有一道题是关于小动物分果子的。
就像在一个美丽的森林里,小松鼠、小兔子和小猴子它们一起找到了好多好多果子。
小松鼠说它先数了数,果子一共有30个。
然后小兔子说它想把果子按照一定的规则分给大家。
它说小松鼠可以分到果子总数的三分之一,小猴子能分到果子总数的五分之一,那剩下的就归自己啦。
这时候我们就要像聪明的小数学家一样,算出小松鼠能拿到几个果子呢?30的三分之一就是10个果子呀。
那小猴子呢,30的五分之一就是6个果子。
这样算下来,小兔子就能得到30 - 10 - 6 = 14个果子啦。
这道题就像是我们在森林里和小动物们一起做游戏,顺便就把数学题给解了。
还有一道题是关于图形的。
就像我们在玩拼图一样。
有一个大的正方形,然后这个正方形被分成了好多小的长方形和三角形。
题目问我们其中一个小三角形的面积是多少。
这就好像我们要把这个大正方形当成一个大蛋糕,然后看看这个小三角形是这个大蛋糕的多少份呢。
我们得先看看这个大正方形的边长是多少,再根据那些长方形和三角形之间的关系,算出小三角形的底和高,这样就能算出面积啦。
就像我们把蛋糕切得整整齐齐的,每一块都能算出大小。
这些真题呀,虽然是考试题目,但是做起来就像在玩游戏一样。
它不会让我们觉得数学很枯燥,反而让我们发现数学在生活中的好多地方都能用到。
就像我们平时和小伙伴分糖果,或者是看看自己的小房间的面积,这都是数学呀。
在做这些真题的时候,我们可能会遇到一些小困难,就像爬山的时候遇到了小石块。
但是只要我们静下心来,像探险家一样勇敢地去探索,就一定能找到答案。
比如说有一道关于数字规律的题,给出了一串数字,让我们找出下一个数字是什么。
一开始可能会觉得很迷糊,但是我们可以一个一个数字去看,就像找宝藏一样,慢慢就会发现数字之间的小秘密。
袋鼠数学数学竞赛试题

袋鼠数学数学竞赛试题
题目,在一个房间里,有一只袋鼠和一只狗。
袋鼠的身高是狗的1/4,袋鼠的体重是狗的1/2。
如果袋鼠的体重增加了20%,那么袋鼠的身高将增加多少?
解答:
1. 利用代数方法解答:
设狗的身高为h,袋鼠的身高为1/4h。
袋鼠的体重为w,狗的体重为2w。
根据题意可得,袋鼠的体重增加20%,即原体重的1.2倍,即1.2w。
设袋鼠身高增加后的身高为x,则有,x = 1/4h + Δh(Δh为身高增加的值)。
根据题意可得,1.2w = 2w (x/h)^3(袋鼠体重的增加与身高的关系)。
整理方程得,(x/h)^3 = 0.6。
解方程可得,x/h ≈ 0.843。
因此,袋鼠的身高增加约为84.3%。
2. 利用比例方法解答:
根据题意可得,袋鼠的身高与狗的身高的比例为1:4,袋鼠的体重与狗的体重的比例为1:2。
设袋鼠的身高增加后的身高为x,根据比例可得,x/h = 1.2。
解方程可得,x = 1.2h。
因此,袋鼠的身高增加了20%。
3. 利用图形方法解答:
设狗的身高为h,袋鼠的身高为1/4h。
袋鼠的体重为w,狗的体重为2w。
根据题意可得,袋鼠的体重增加20%,即原体重的1.2倍,即1.2w。
画出狗和袋鼠的身高和体重的比较图,可以观察到袋鼠的身高增加了20%后,狗和袋鼠的身高之间的比例关系仍然保持不变。
综上所述,袋鼠的身高增加了约84.3%。
袋鼠数学数学题

袋鼠数学题,也被称为“袋鼠数学竞赛”,是一个面向中
学生的数学竞赛,其题目以问题解决和数学应用为主。
以下
是一份袋鼠数学竞赛的样题:
选择题
1. 如果一只袋鼠一年跳300次,那么五只袋鼠跳多少次?
A. 1500次
B. 1400次
C. 1300次
D. 1200次
E. 1100次
2. 在一个等边三角形中,一个顶点到一个对边的中点的
距离是3米,那么这个三角形的边长是多少?
A. 3米
B. 4米
C. 5米
D. 6米
E. 8米
3. 一个圆和一个扇形的半径相等。
已知圆的面积是50平
方厘米,而扇形所在圆的面积是200平方厘米,这个扇形的
圆心角是多少度?
A. 72度
B. 108度
C. 180度
D. 360度
E. 720度
答案:A,C,B。
解释:第一题根据比例关系计算;第二题利用勾股定理计算;第三题根据面积比计算圆心角。
通过这些题目可以看出,袋鼠数学竞赛的题目强调数学的应用和问题解决能力,而不仅仅是理论知识和计算能力。
因此,想要在袋鼠数学竞赛中取得好成绩,需要具备广泛的数学知识、敏锐的观察力和良好的思维习惯。
袋鼠思维数学竞赛报名历年题目

选择题在袋鼠思维数学竞赛中,若一个等差数列的前n项和为S_n,且S_3 = 6,S_6 = 21,则S_9等于:A. 45B. 54(正确答案)C. 63D. 72竞赛题目:设f(x) = x3 - 3x2 + 2x,则f(f(x)) = 0的实数根个数为:A. 3B. 4C. 5(正确答案)D. 6袋鼠思维数学竞赛中,若一个直角三角形的两条直角边长度分别为a和b,且满足a2 + b2 = 100,c为斜边,则c的取值范围是:A. (0, 10)B. [10, +∞)C. (10, 10√2](正确答案)D. [10√2, +∞)竞赛中,若一个圆的半径为r,内接于一个边长为a的正三角形中,则该圆的面积与正三角形面积之比为:A. π/3B. π/4C. π/(3√3)(正确答案)D. π/6在袋鼠思维数学竞赛的数列问题中,若数列{a_n}满足a_1 = 1,a_{n+1} = a_n + 2n,则a_10等于:A. 81B. 90C. 99D. 100(正确答案)竞赛题目:若一个长方体的长、宽、高分别为a、b、c,且满足a + b + c = 6,则长方体的体积V的最大值为:A. 8B. 27/8C. 27/4D. 27(正确答案)在袋鼠思维数学竞赛的几何问题中,若一个等腰三角形的底边长为2a,底角为θ,则三角形的面积S关于θ的表达式为:A. a2sin(θ)B. a2cos(θ)C. a2sin(2θ)/2(正确答案)D. a2cos(2θ)/2竞赛中,若一个函数的图像在x轴上方,且其导函数在x=0处取得极小值,则该函数在x=0处:A. 一定有拐点B. 一定有极值点C. 可能有拐点也可能有极值点(正确答案)D. 既无拐点也无极值点在袋鼠思维数学竞赛的组合数学问题中,从1到9的九个数字中任选三个不同的数字,组成一个没有重复数字的三位数,且这个三位数是3的倍数,这样的三位数共有多少个?A. 120B. 168(正确答案)C. 216D. 288。
2004年加拿大数学奥林匹克

2. How many ways can 8 mutually non-attacking rooks be placed on the 9 × 9 chessboard (shown here) so that all 8 rooks are on squares of the same colour? [Two rooks are said to be attacking each other if they are placed in the same row or column of the board.]
Solution 2 Consider rooks on black squares first. We have 8 rooks and 9 rows, so exactly one row will be without rooks. There are two cases: either the empty row has 5 black squares or it has 4 black squares. By permutation these rows can be made either last or second last. In each case we’ll count the possible number of ways of placing the rooks on the board as we proceed row by row. In the first case we have 5 choices for the empty row, then we can place a rook on any of the black squares in row 1 (5 possibilities) and any of the black squares in row 2 (4 possibilities). When we attempt to place a rook in row 3, we must avoid the column containing the rook that was placed in row 1, so we have 4 possibilities. Using similar reasoning, we can place the rook on any of 3 possible black squares in row 4, etc. The total number of possibilities for the first case is 5 · 5 · 4 · 4 · 3 · 3 · 2 · 2 · 1 = (5!)2 . In the second case, we have 4 choices for the empty row (but assume it’s the second last row). We now place rooks as before and using similar logic, we get that the total number of possibilities for the second case is 4 · 5 · 4 · 4 · 3 · 3 · 2 · 1 · 1 = 4(5!4!). Now, do the same for the white squares. If a row with 4 white squares is empty (5 ways to choose it), then the total number of possibilities is (5!)2. It’s impossible to have a row with 5 white squares empty, so the total number of ways to place rooks is (5!)2 + 4(5!4!) + (5!)2 = (5 + 4 + 5)5!4! = 14(5!4!).
袋鼠数学历年试题

袋鼠数学历年试题今天咱们来聊聊袋鼠数学的历年试题呀。
袋鼠数学竞赛可有趣啦。
它的试题就像一个个小挑战,等着我们去攻克。
我做过一些历年的试题,那里面的题目可真是五花八门。
比如说有一道关于小动物分果子的题目。
就像在一个大森林里,小兔子有一堆果子,它要分给小松鼠和小猴子一些。
题目里告诉我们小兔子有多少个果子,小松鼠想要几个,小猴子又想要几个。
然后问我们小兔子还剩下几个果子呢。
这就像我们平时和小伙伴分小零食一样呀。
我当时就想着我和我的好朋友分糖果的情景,就很容易算出答案啦。
还有那种关于图形的题目。
就像我们在玩拼图游戏。
有一个大的图形,然后又给了我们几个小的图形,问我们怎么把小图形拼到大图形里面去呢。
我记得有一次是一个像房子形状的大图形,小图形有三角形、正方形。
我就想象着我在搭积木盖房子,把那些小图形一块一块地放在合适的位置。
这让我觉得做试题就像在玩游戏一样。
在历年试题里,也有关于数字排队的题目。
就好比小动物们排队领东西,数字们也要排队。
它会告诉我们一些数字的位置,然后问另一个数字应该在什么地方。
我想到了我们在学校排队做早操,我站在某个同学后面,那另一个同学又该站哪里呢?这样想的话,题目就变得很简单了。
这些试题不仅仅是为了考试,还让我们学会用有趣的方式思考数学问题。
我每次做完一些试题,就感觉自己像一个聪明的小探险家,又发现了数学世界里的一个小秘密。
而且当我做对一道题的时候,那种开心就像我在学校跑步比赛得了第一名一样。
虽然有时候也会遇到很难的题目,就像爬山遇到很陡的坡。
但是只要我们不放弃,再仔细看看题目,就像在山上找另外的小路一样,总会找到解决的办法的。
我特别喜欢做袋鼠数学的历年试题,因为它让数学变得不再那么枯燥。
你们要是有机会做这些试题,一定会像我一样,发现数学原来是这么好玩的东西呢。
它就像一个充满惊喜的大盒子,每一道试题都是一个小惊喜在等着我们去打开。
加拿大国际袋鼠数学竞赛试题-2013年

(A) 12
(B) 8
(C) 4
(D) 2
(E) 0
3. Which of the dresses has less than seven dots, but more than five dots?
(A)
(B)
(C)
(D)
(E)
Grade 1-2
Year 2013
4. A lot of babies were born in the zoo last year: two baby lions, three baby dolphins and four baby eagles.
tulips. They started at 9:00 in the morning. At what time will they finish planting all 20 tulips?
(A) At 9:10
(B) At 9:20 (C) At 9:40
(D) At 9:50
(E) At 10:00
(C) 2 and 0
(D) 6 and 9
(E) 7 and 1
2. There are twelve books on a shelf and four children in a room. How
many books will be left on the shelf if each child takes one book?
(A) 16
(B) 30
(C) 50
(D) 52
(E) 70
18. In a park there are babies in four-wheel strollers and children on two-wheel bikes. Paula counted wheels and the total was 12. When she added the number of strollers to the number of bikes, the total was 4. How many two-wheel bikes are there in the park?
袋鼠数学数学竞赛中文试题

袋鼠数学数学竞赛中文试题袋鼠数学数学竞赛中文试题Ⅰ.选择题(每题2分,共10分)1. 下列哪个数是一个素数?A. 25B. 31C. 42D. 502. A、B、C三个人分别携带了2本、3本、5本书,他们总共带了多少本书?A. 6B. 10C. 9D. 73. 一些苹果在3个篮子中平均分配,每个篮子得到10个苹果,若再将这些苹果平均分配到6个篮子中,则每个篮子得到多少个苹果?A. 5B. 10C. 15D. 204. 甲、乙、丙三个人分别花费400元、600元、800元购买了一些物品,他们所花费的总金额是多少元?A. 800B. 1200C. 1800D. 16005. 若9+4x=25,则x的值是多少?A. 4B. 3C. 5D. 2Ⅱ.填空题(每题3分,共15分)1. 一个整数减去两个负整数之和能是正整数吗?为什么?________________________________________________2. 一个多边形的内角和是2160°,这个多边形有多少个角?________________________________________________3. 甲、乙两个容器分别装有2升和3升的水,如何只用这两个容器倒水,可以得到1升的水?________________________________________________4. 如果一个数的平方加上这个数的2倍等于18,求出这个数。
________________________________________________5. 某树在一年内的生长长度是150厘米,第一季度它的生长长度是前两个季度长度之和的1.5倍,第二季度它的生长长度是前两个季度长度之和的0.5倍,求出第三季度它的生长长度。
________________________________________________Ⅲ.解答题(每题10分,共30分)1. 中国的国旗是由什么颜色组成的?每种颜色的面积占比是多少?________________________________________________2. 一辆火车从A站出发,以每小时100千米的速度前进,过了1小时到达B站。
袋鼠数学国际数学竞赛题

袋鼠数学国际数学竞赛题摘要:1.代码外提的背景和意义2.代码外提的基本概念3.代码外提的实践方法和技巧4.代码外提的实际应用案例5.代码外提的未来发展趋势和挑战正文:一、代码外提的背景和意义随着互联网技术的飞速发展,软件开发行业也迎来了黄金时期。
在这个过程中,代码质量、开发效率和协同能力成为了软件开发团队的核心竞争力。
为了满足市场需求,提高软件开发的效率,代码外提应运而生。
代码外提,即提取代码中的关键部分,将其独立为一个模块或者函数,以实现代码复用和优化。
二、代码外提的基本概念代码外提主要包括以下几个方面的内容:1.提取函数:将重复出现的代码片段提取为函数,以实现代码复用。
2.模块化:将复杂的项目结构进行模块化处理,提高代码的可读性和可维护性。
3.抽象:将具体实现抽象为接口或者抽象类,降低模块间的耦合度。
三、代码外提的实践方法和技巧进行代码外提时,可以采用以下方法和技巧:1.识别重复代码:通过代码审查、静态分析等手段,找出重复出现的代码片段。
2.选择合适的抽象层次:根据项目的需求和架构,选择合适的抽象层次,如接口、抽象类等。
3.优化命名规范:遵循命名规范,提高代码的可读性。
4.编写详尽的注释:为提取的代码添加详细的注释,方便其他开发者理解和使用。
四、代码外提的实际应用案例代码外提在实际项目中的应用案例如下:1.提取登录验证功能:将登录验证功能从主函数中提取为一个独立的函数,实现代码复用。
2.模块化处理:将一个大型项目按照功能模块进行划分,提高项目的可读性和可维护性。
3.抽象为接口:将具体的数据操作类抽象为接口,降低不同模块间的耦合度。
五、代码外提的未来发展趋势和挑战随着软件开发技术的不断发展,代码外提将面临以下趋势和挑战:1.自动化:借助人工智能、机器学习等技术,实现代码外提的自动化。
2.智能化:结合代码分析工具,提供更智能的代码外提建议。
3.挑战:如何在保证代码外提质量的同时,提高开发效率和协同能力,将是一个长期的挑战。
袋鼠数学竞赛题目

袋鼠数学竞赛题目
袋鼠数学竞赛是一项全球规模最大的青少年数学竞赛,针对1-12年级的学生。
这个竞赛的题目相比其他的数学竞赛题更具趣味性,对孩子来说也是练手的好机会。
以下是一些题目的例子:
阅读理解题:这是1、2年级的题目,你让孩子读一下,看看能否看得懂题目?第一遍看这个题目的时候有点懵,再仔细看题目、看图案,才发现,原来每只瓢虫身上都有圆点,而圆点的数量也各不一样,因此文中强调了一句“in the order of increasing number of dots”,必须要理解这句话,才能明白需要根据圆点的数量来连接瓢虫的道理。
这题的答案是D。
视觉训练题:看下面这道1、2年级的视觉训练题目,问一个图像颜色交换一下后会变成什么样子?答案是E。
等到了3、4年级,还是同样的图案,但是对孩子思维难度要求更高。
你看下面的题目,不仅仅是需要孩子将颜色交换一下,还需要将图像旋转一下,问最后变成什么样子?答案选E。
建模能力题:我们看下面这道3、4年级的题目,有4个球,分别是10g、20g、30g和40g,根据图里面的天平指示,问哪个球是30g?这道题目就需要孩子能根据图像所示建立一个数学模型,否则这道题目TA是做不出来的!这个数学模型应该是下面这个样子:第一个模型是:A+B > C+D,这对应于第一张图,表示A和B的重量比C和D的重量重。
第二个模型是:B+D = C。
这对应于第二张图,表示B和D的重量和C一样重。
有了这两个模型,孩子把数字带入进去,就比较容易得到答案了!答案是C。
袋鼠数学竞赛历年真题中文

袋鼠数学竞赛历年真题中文一、2008年1、给定4个正整数a,b,c,d,请解决:$$\frac{a}{b}+\frac{c}{d}=?$$2、证明:若正整数m,n满足$m \cdot n= 85$,则$m + n \le 19$二、2009年1、圆锥曲线的方程为$${x^2} + {y^2} = 16{x^2},$$试求它的渐近线的方程?2、已知正方形ABCD的面积为36,点E在BC边上,DE=4。
求正三角形ABE的面积?三、2010年1、设$a,b \in R$,试证明下列结论:若$a^2+b^2=1$,$a \ne 0$,则$\frac{1}{a}+\frac{1}{b} \ge 2$2、三棱锥的一边角为$\frac{\pi }{3}$,其余直角三角形的斜边长分别为1,2,3,求这个三棱锥的体积。
四、2011年1、在正四面体ABCD中,AB=a,BC=b,AD=c,则其表面积为____2、若$a$,$b$,$c$为不相等的正数,$a+b+c=1$,请证明:$a^3+b^3+c^3 \ge abc$五、2012年1、设$m, n$是正整数,$m \ge n$,试证明:$m+n \le m^2-mn+n^2$2、设正方形$ABCD$中,$B(-3,2)$,$AD=8$,试求$ABCD$的外接圆的方程?六、2013年1、求函数$f(x)=x^2(x-1)^2$的最大值?2、求$\frac{1}{2}x(x+1)(x-1)$的三个零点的和?七、2014年1、已知变量$x,y$满足$x+y=100$,试求$f(x,y)=20x^2-44xy+90y^2$的最大值?2、设函数$f(x)=\frac{1}{{1 + 2x}} + 3e^x$的定义域为$[2,3]$,试求$f(x)$在定义域中的最小值?八、2015年1、若$x,y,z\in R^+$,满足$x^2+y^2+z^2=14$,求证:$xy+yz+zx \ge 6\sqrt {3}$2、若$ab+bc+ca=36,a \ge b \ge c$,求$a,b,c$的值?九、2016年1、求函数$y=\frac{x^2+15x+50}{x^2+10x+25}$的零点?2、若$a,b,c$满足$2a^2+b+c=15$, $b+c\ge 9$,求证:$bc \ge 6$?十、2017年1、若$3x^2+2xy+7y^2-13xy=0$,求$x,y$的最大值?2、圆锥曲线$x^2+y^2=16x^2$的双曲线半径为___?。
加拿大国际袋鼠数学竞赛试题-2013年

加拿⼤国际袋⿏数学竞赛试题-2013年Grade 1-2International Contest-Game MATH KANGAROOPart A: Each correct answer is worth 3 points. 1. Which digits are missing?Year 2013(A) 3 and 5 (B) 4 and 8(C) 2 and 0(D) 6 and 9(E) 7 and 12. There are twelve books on a shelf and four children in a room. Howmany books will be left on the shelf if each child takes one book?(A) 12(B) 8(C) 4(D) 2(E) 03. Which of the dresses has less than seven dots, but more than five dots?(A)(B)(C)(D)(E)Grade 1-2Year 20134. A lot of babies were born in the zoo last year: two baby lions, three baby dolphins and four baby eagles. How many legs do all these babies have altogether?(A) 20(B) 18(C) 16(D) 14(E) 125. Several students want to plant 20 tulips in the school garden. It takes ten minutes for them to plant five tulips. They started at 9:00 in the morning. At what time will they finish planting all 20 tulips?(A) At 9:10(B) At 9:20 (C) At 9:40(D) At 9:50(E) At 10:006. How many more bricks are there in the larger stack?(A) 4(B) 5(C) 6(D) 7Part B: Each correct answer is worth 4 points.(E) 107. Ann has. Barb gave Eve. Jim has. Bob has. Who is Barb?(A)(B)(C)8. There is a path with square tiles.(D)(E)How many tiles fit in the area inside?(A) 5(B) 6(E) 9Grade 1-2Year 20139. Cat and Mouse are moving to the right. When Mouse jumps 1 tile, Cat jumps 2 tiles at the same time.On which tile does Cat catch Mouse?(A) 1(B) 2(C) 3(D) 4(E) 510. I am a number. If you count by tens you will say my name. I am not ten. If you add me to 30, you will get anumber less than 60. Who am I?(A) 20(B) 30(C) 40(D) 50(E) 6011. There is a house on each corner of the streets. The housesare shown on the map. Two new houses will be built oneach street between the corner houses. How many houseswill there be in all?(A) 8(B) 12(C) 16(D) 20(E) Other answer12. Kasia has 3 brothers and 3 sisters. How many brothers and how many sisters does her brother Mike have?(A) 3 brothers and 3 sisters(B) 3 brothers and 4 sisters(C) 2 brothers and 3 sisters(D) 3 brothers and 2 sisters(E) 2 brothers and 4 sistersPart C: Each correct answer is worth 5 points.13. Ania makes a large cube from 27 small white cubes. She paints all the faces of the large cube. Then Ania removes four small cubes from four of the corners, as shown. While the paint is still wet, she stamps each of the new faces onto a piece of paper. How many of the following stamps can Ania make?(A) 1(B) 2(C) 3(D) 4(E) 514. Ann has a lot of these pieces:She tries to put them in the square, as many as possible. How many cells shall be left empty?(A) 0(B) 1(C) 2(D) 3(E) 4Grade 1-215. In a game it is possible to make the following exchanges:Year 2013Adam has 6 pears. How many strawberries will Adam have, when he trades all his pears for juststrawberries?(A) 12(B) 36(C) 1816. Sophie makes a row of 10 houses with matchsticks. In the picture you can see the beginning of the row. How many matchsticks does Sophie need altogether?(A) 50(B) 51(C) 55(D) 60(E) 6217. A square box is filled with two layers of identical square pieces of chocolate. Kirill has eaten all 20 pieces in the upper layer, which are along the walls of the box. How many pieces of chocolate are left in the box?(A) 16(B) 30(C) 50(D) 52(E) 7018. In a park there are babies in four-wheel strollers and children on two-wheel bikes. Paula counted wheels and the total was 12. When she added the number of strollers to the number of bikes, the total was 4. How many two-wheel bikes are there in the park?(A) 1(B) 2(C) 3(D) 4(E) Other numberGrade 3-4Year 2013International Contest-Game MATH KANGAROOPart A: Each correct answer is worth 3 points. 1. In which figure is the number of black kangaroos bigger than the number of white kangaroos?(A)(B)(C)(D)(E)2. Aline writes a correct calculation. Then she covers two digits which are the same with a sticker:Which digit is under the stickers?(A)(B)(C)(D)(E)3. Monica arrived in the Kangaroo Camp on July 25th in the morning and left the camp on August 3rd inthe afternoon. How many nights did she sleep in the camp?(A) 7(B) 9(C) 10(D) 30(E) 84. How many triangles of all sizes can be seen in the picture below?(A) 9(B) 10(C) 11(D) 13(E) 125. In London 2012, the USA won the most medals: 46 gold, 29 silver and 29 bronze. China was secondwith 38 gold, 27 silver and 23 bronze. How many more medals did the USA win compared to China?(A) 6(B) 14(C) 16(D) 24Grade 3-4Year 20136. There are three families in my neighbourhood with three children each; two of the families havetwins. All twins are boys. At most how many girls are in these families?(A) 2(B) 3(C) 4(D) 5(E) 67. Vero's mother prepares sandwiches with two slices of bread each. A package of bread has 24 slices.How many sandwiches can she prepare from two and a half packages of bread?(A) 24(B) 30(C) 48(D) 34(E) 268. About the number 325, five boys said:Andrei: "This is a 3-digit number"Boris: "All digits are distinct"Vick: "The sum of the digits is 10"Greg: "The units digit is 5"Danny: "All digits are odd"Which of the boys was wrong?(A) Andrei(B) Boris(C) Vick(D) Greg(E) DannyPart B: Each correct answer is worth 4 points. 9. The rectangular mirror was broken.Which of the following pieces is the missing part of the broken mirror?(A)(B)(C)(D)(E)10. When Pinocchio lies, his nose gets 6 cm longer. When he tells the truth, his nose gets 2 cm shorter. When his nose was 9 cm long, he told three lies and made two true statements. How long was Pinocchio's nose afterwards?(A) 14 cm(B) 15 cm(C) 19 cm(D) 23 cm(E) 31 cmGrade 3-4Year 201311. John is 33 years old. His three sons are 5, 6 and 10 years old. In how many years will the three sons together be as old as their father?(A) 4(B) 6(C) 8(D) 10(E) 1212. On the map, white lines represent streets. There are pictograms on some intersections (for example, trafic light, basket, tram). Ann started walking at the beginning of the middle vertical street in the direction of the arrow. At every intersection of streets she turned either to the right or to the left. First she turned right, then left, then again left, then right, then left, and finally again left. Which of the landmarks did Ann approach in the end?(A)(B)(E)13. Schoolmates Andy, Betty, Cathie and Dannie were born in the same year. Their birthdays were on February 20th, April 12th, May 12th and May 25th, not necessarily in this order. Betty and Andy were born in the same month. Andy and Cathie were born in the same day of different months. Who of these schoolmates is the oldest?(A) Andy(B) Betty(C) Cathie (D) Dannie (E) impossible to determine14. In the Adventure Park, 30 children took part in two of the adventures. 15 of them participated in the "moving bridge" contest, and 20 of them went down the zip-wire. How many of the children took part in both adventures?(A) 25(B) 15(C) 30(D) 10(E) 515. Which of the five pieces in the answers fits with the piece in the separate picture, so that together they form a rectangle?(A)(B)(C)(D)(E)16. Children in the school club had to arrange fitness balls according to their size from the biggest to the smallest one. Rebecca was comparing them and said: the red ball is smaller than the blue one, the yellow one is bigger than the green one, and the green one is bigger than the blue one. What is the correct order of the fitness balls?(A) green, yellow, blue, red (D) yellow, green, blue, red(B) red, blue, yellow, green (E) blue, yellow, green, red(C) yellow, green, red, blueGrade 3-4Year 2013Part C: Each correct answer is worth 5 points.17. In the shown triangle, first we join the midpoints of all the three sides. This way, we form a smaller triangle. We repeat this one more time with the smaller triangle, forming a new even smaller triangle, which we colour in red. How many triangles of the size of the red triangle are needed to cover completely the original triangle, without overlapping?Note: Midpoint of a side is the point that divides the side in two parts of the same length.(A) 5(B) 8(C) 10(D) 16(E) 3218. There are oranges, apricots and peaches in a big basket. How many fruits are there in the basket if the peaches and the apricots together are 18, the oranges and the apricots together are 28 and 30 fruits are not apricots?(A) 46(B) 20(C) 40(D) 38(E) 2919. In December Tom-the-cat slept for exactly 3 weeks. Which calculations should we do in order to find how many minutes he stayed awake during this month?(A) (31 – 7) × 3 × 24 × 60(B) (31 – 7 × 3) × 24 × 60(C) (30 – 7 × 3) × 24 × 60(D) (31 – 7 ) × 24 × 60(E) (31 – 7 × 3) × 24 × 60 × 6020. Basil has several domino tiles, as shown in the figure. He wants to arrange them in a line according to the well-known "domino rule": in any two tiles that are next to each other, the squares that touch must have the same number of points. What is the largest number of tiles he can arrange in this way?(A) 3(B) 4(C) 521. Cristi has to sell 10 glass bells that vary in price: 1 euro, 2 euro, 3 euro, 4 euro, 5 euro, 6 euro, 7 euro, 8 euro, 9 euro, 10 euro. In how many ways can Cristi divide all the glass bells in three packages so that all the packages have the same price?(A) 1(B) 2(C) 3(D) 4(E) Such a division is not possible.Grade 3-4Year 201322. Nancy bought 17 cones of ice-cream for her three children. Misha ate twice as many cones as Ana. Dan ate more ice-cream than Ana but less than Misha. How many cones of ice-cream did Dan eat?(A) 4(B) 5(C) 6(D) 7(E) 823. Peter bought a carpet 36 dm wide and 60 dm long. The figure shows part of this carpet. As seen, the carpet has a pattern of small squares containing either a sun or a moon. You can count that along the width there are nine squares. When the carpet is fully unrolled, how many moons will be seen?(A) 68(B) 67(C) 65(D) 63(E) 6024. Beatrice has a lot of pieces like the grey one in the picture. At least how many of these grey pieces will she need to makea grey square?(A) 3(B) 4(C) 6(D) 8(E) 16Grade 11-12International Contest-Game MATH KANGAROOPart A: Each correct answer is worth 3 points.Year 20131. Which of the following numbers is the largest?(A) 2013(B) 20+13(C) 2013(D) 2013(E) 20 × 132. Four circles of radius 1 are touching each other and a smaller circle as seen in the picture. What is the radius of the smaller circle?(A) 2 ?11 (B)23 (C)43 (D)47 (E)163. A three-dimensional object bounded only by polygons is called a polyhedron. What is the smallestnumber of polygons that can bind a polyhedron, if we know that one of the polygons has 12 sides?(A) 12(B) 13(E) 244. The cube root of 333 is equal to(A) 33(B) 333 ?1(C) 323(D) 332(E) ( 3)35. The year 2013 has the property that its number is made up of the consecutive digits 0, 1, 2 and 3.How many years have passed since the last time a year was made up of four consecutive digits?(A) 467(B) 527(C) 581(D) 693(E) 9906. Let f be a linear function for which f(2013) – f(2001) = 100. What is f(2031) – f(2013)?(A) 75(B) 100(C) 120(D) 150(E) 1807. Given that 2 < x < 3, how many of the following statements are true?4 < x2 < 94 < 2x < 96 < 3x < 9 0 < x2 ? 2x < 3(A) 0(B) 1(C) 2(D) 3(E) 48. Six superheroes capture 20 villains. The first superhero captures one villain, the second capturestwo villains and the third captures three villains. The fourth superhero captures more villains thanany of the other five. What is the smallest number of villains the fourth superhero must havecaptured?(A) 7(B) 6(C) 5(D) 4(E) 3Grade 11-12Year 20139. In the cube to the right you see a solid, non-transparent pyramid ABCDS with base ABCD, whose vertex S lies exactly in the middle of an edge of the cube. You look at this pyramid from above, from below, from behind, from ahead, from the right and from the left. Which view does not arise?(A)(B)(C)(D)(E)10.Whena certainsolid substancemelts,itsvolume increasesby1 12.By how much doesitsvolumedecrease when it solidifies again?(A)1 10(B)1 11(C)1 12(D)1 13(E)1 14Part B: Each correct answer is worth 4 points.11. The diagram shows two squares of equal side length placed so thatthey overlap. The squares have a common vertex and the sides make anangle of 45 degrees with each other, as shown. What is the area of theoverlap as a fraction of the area of one square?1 (A)21 (B)2(C) 1? 1 2(D) 2 ?12 ?1 (E)212.How many positive integers n exist such that bothn 3and 3nare three-digit integers?(A) 12(B) 33(C) 34(D) 100(E) 30013. A circular carpet is placed on a floor of square tiles. All the tiles which have more than one point in common with the carpet are marked grey. Which of the following is an impossible outcome?(A)(B)(C)(D)(E)14. Consider the following statement about a function f on the set of integers: "For any even x, f(x) is even." What would be the negation of this proposition?(A) For any even x, f(x) is odd(B) For any odd x, f(x) is even(C) For any odd x, f(x) is odd(D) There exists an even number x such that f(x) is odd(E) There exists an odd number x such that f(x) is oddGrade 11-12Year 201315. How many pairs (x,y) of positive integers satisfy the equation x2 y3 = 612 ?(A) 6(B) 8(C) 10(D) 12(E) Another number.16. Given a function W (x) = (a ? x)(b ? x)2 , where a < b. Its graph is in one of the following figures. In which one?(A)(B)(C)(D)(E)17. Consider a rectangle, one of whose sides has a length of 5. The rectangle can be cut into a squareand a rectangle, one of which has the area 4. How many such rectangles exist?(A) 1(B) 2(C) 3(D) 4(E) 518. Assume that x2 ? y2 = 84 , where x and y are positive integers. How many values may theexpression x2 + y2 have?(A) 1(B) 2(C) 3(D) 5(E) 619. In the triangle ABC the points M and N on the side AB are such that AN = ACand BM = BC. Find ∠ACB if ∠MCN = 43°.(A) 86°(B) 89°(C) 90°(D) 92°(E) 94°20. A box contains 900 cards numbered from 100 to 999. Any two cards have different numbers.Fran?ois picks some cards and determines the sum of the digits on each of them. At least how manycards must he pick in order to be certain to have three cards with the same sum?(A) 51(B) 52(C) 53(D) 54(E) 55Part C: Each correct answer is worth 5 points.21. How many pairs (x,y) of integers with x ≤ y exist such that their product equals 5 times their sum?(A) 4(B) 5(C) 6(D) 7(E) 822. Let f (x), x ∈ R be the function defined by the following properties: f is periodic with period 5 andf (x) = x2 when x ∈[?2,3) . What is f(2013) ?(A) 0(B) 1(C) 2(D) 4(E) 923. We have many white cubes and many black cubes, all of the same size. We want to build a rectangular prism composed by exactly 2013 of these cubes so that they are placed alternating a white cube and a black cube in all directions. If we start putting a black cube in one of the eight corners of the prism, how many black squares will we see on the exterior surface of the solid?(A) 887(B) 888(C) 890(E) It depends on the dimensions of the prism(D) 892Grade 11-12Year 201324. How many solutions (x,y), where x and y are real numbers, does the equation x2 + y2 = x + yhave? (A) 1(B) 5(C) 8(D) 9(E) Infinitely many.25. There are 2013 points marked inside a square. Some of them are connected to the vertices of thesquare and with each other so that the square is divided into non-overlapping triangles. All markedpoints are vertices of these triangles. How many triangles are formed this way?(A) 2013(B) 2015(C) 4026(D) 4028(E) impossible to determine26. There are some straight lines drawn on the plane. Line a intersects exactly three other lines and lineb intersects exactly four other lines. Linec intersects exactly n other lines, with n ≠ 3, 4 .Determine the number of lines drawn on the plane.(A) 4(B) 5(C) 6(D) 7(E) Another number.27. The sum of the first n positive integers is a three-digit number in which all of the digits are thesame. What is the sum of the digits of n?(A) 6(B) 9(C) 12(D) 15(E) 1828. On the island of Knights and Knaves there live only two types of people: Knights (who always speak the truth) and Knaves (who always lie). I met two men who lived there and asked the taller man if they were both Knights. He replied, but I could not figure out what they were, so I asked the shorter man if the taller was a Knight. He replied, and after that I knew which type they were. Were the men Knights or Knaves?(A) They were both Knights.(B) They were both Knaves.(C) The taller was a Knight and the shorter was a Knave.(D) The taller was a Knave and the shorter was a Knight.(E) Not enough information is given.29. Julian has written an algorithm in order to create a sequence of numbers as a1 = 1,am+n = am + an + mn , where m and n are natural numbers. Find the value of a100.(A) 100(B) 1000(C) 2012(D) 4950(E) 505030. The roundabout shown in the picture is entered by 5 cars at the same time, eachone from a different direction. Each of the cars drives less than one round and notwo cars leave the roundabout in the same direction. How many differentcombinations are there for the cars leaving the roundabout?(A) 24(B) 44(C) 60(D) 81(E) 120Year 2013Grade 1 and 2 DBACCB DEDABE DACBDBGrade 3 and 4 DDBBCDBE BDBADEBD DDBCEBBBGrade 5 and 6 ECCBEBBECD CCDBADDACD ADBABDBBDBGrade 7 and 8 DBACEECEAC DEBCBAABBC AEDCCABDBCGrade 9 and 10 DBCCBAECBC DBDADDBCEB DCCEEDCCBBGrade 11 and 12 CABDCDEBED DAEDEADBEC ADCED*CBDEB*Answer E was also accepted as correct for Q25 Answers。
加拿大国际袋鼠数学竞赛试题 -2008年
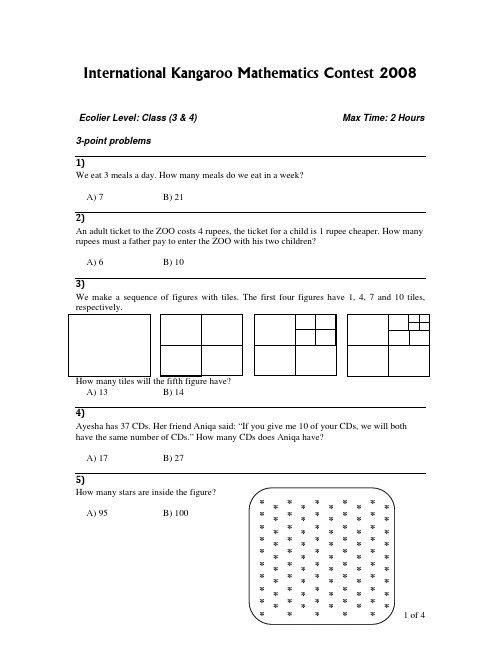
International Kangaroo Mathematics Contest 2008Ecolier Level: Class (3 & 4)Max Time: 2 Hours3-point problems1)We eat 3 meals a day. How many meals do we eat in a week?A) 7 B) 212)An adult ticket to the ZOO costs 4 rupees, the ticket for a child is 1 rupee cheaper. How many rupees must a father pay to enter the ZOO with his two children?A) 6 B) 10 3)We make a sequence of figures with tiles. The first four figures have 1, 4, 7 and 10 tiles, respectively.How many tiles will the fifth figure have? A) 13 B) 144)Ayesha has 37 CDs. Her friend Aniqa said: “If you give me 10 of your CDs, we will both have the same number of CDs.” How many CDs does Aniqa have?A) 17 B) 27 5)How many stars are inside the figure?A) 95 B) 100Rabia has drawn a point on a piece of paper. She now draws four straight lines that pass through this point. Into how many sections do these lines divide the paper?A) 4 B) 87)In six and one half hours it will be four hours after midnight. What time is it now?A) 21:30 B) 10:308)The storm made a hole in the front side of the roof. There were 10 roof tiles in each of 7 rows. How many tiles are left on the front side of the roof?A) 57 B) 599)Ejaz is making figures with two triangular cards shown. Which figure he cannot get?A) B)Ahmad multiplies by 3, Nasir adds 2, and Tahir subtracts 1. In what order can they do this to convert 3 into 14?A) Ahmad, Nasir, Tahir B) Nasir, Ahmad, Tahir11)Usman is taller than Noman and shorter than Salman. Who is the tallest?A) Usman B) Salman12)Abida made the figure on the right out of five cubes. Which ofthe following figures (when seen from any direction) can shenot get from the figure on the right side if she is allowed tomove exactly one cube?A) B)13)Which of the following figures is shown most often in the above sequence?B) All of them are shown equally often14)In a hotel, how many two-bed rooms should be added to 5 three-bed rooms to host 21 guests?A) 3 B) 615)There are three songs on a CD. The first song is 6 minutes and 25 seconds long, the second song is 12 minutes and 25 seconds long, and the third song is 10 minutes and 13 seconds long. How long are all the three songs together?A) 29 minutes 3 seconds B) 31 minutes 13 seconds16)We have a large number of blocks of 1 x 2 x 4 cm. We will try to put as many of these blocks as possible in a box of 4 x 4 x 4 cm so that we can close the box with a lid. How many blocks fit in?A) 8 B) 1017)Shaheen shoots two arrows at the target. In the drawing we see that her score is 5. If both arrows hit the target, how many different scores can she obtain?A) 6 B) 318)A garden in the shape of a square is divided into a pool (P) a flowerbed (F) a lawn (L) and a sandpit (S) (see the picture). The lawn and the flowerbed are in the shape of a square. The perimeter of the lawn is 20 m, the perimeter of the flowerbed is 12 m. What is the perimeter of the pool?A) 12 m B) 16 m19)Zahid has as many brothers as sisters. His sister Zahida has twice as many brothers as she has sisters. How many children are there in this family?A) 3 B) 7 20)How many two-digit numbers are there in which the digit on the right is larger than the digit on the left?A) 26 B) 36_______________________________GOOD LUCK !。
数学竞赛袋鼠试题及答案

数学竞赛袋鼠试题及答案试题一:小明有5个苹果,他决定将它们平均分给3个朋友。
如果每个朋友得到的苹果数量相等,那么每个朋友会得到多少苹果?答案:小明有5个苹果,要平均分给3个朋友。
5除以3等于1余2。
所以,每个朋友可以得到1个苹果,剩下2个苹果无法平均分配。
试题二:一个长方形的长是宽的两倍,如果长是10厘米,那么这个长方形的面积是多少?答案:长方形的长是宽的两倍,所以宽是10除以2,等于5厘米。
长方形的面积是长乘以宽,即10厘米乘以5厘米,等于50平方厘米。
试题三:如果一个数的平方等于这个数本身,那么这个数可以是什么?答案:一个数的平方等于这个数本身,这个数可以是0或1。
因为0的平方是0,1的平方是1。
试题四:在一个圆中,半径增加了10%,那么圆的面积增加了多少百分比?答案:设原圆的半径为r,增加后的半径为1.1r。
原圆的面积为πr²,新圆的面积为π(1.1r)²=1.21πr²。
面积增加了(1.21πr² - πr²) / πr² = 0.21,即增加了21%。
试题五:一个班级有40名学生,如果每个学生都至少参加一个兴趣小组,并且每个兴趣小组最多只能有10名学生,那么至少需要多少个兴趣小组?答案:如果每个兴趣小组最多有10名学生,那么40名学生至少需要40/10=4个兴趣小组。
但是,如果每个学生都至少参加一个兴趣小组,那么至少需要5个兴趣小组,因为4个兴趣小组只能容纳40名学生,而最后一个兴趣小组至少需要1名学生。
结束语:以上是数学竞赛袋鼠试题及答案,希望这些题目能够帮助你更好地理解数学问题,并提高解题能力。
数学是一种美妙的语言,通过不断的练习和思考,你将能够发现它的魅力。
袋鼠数学竞赛试题及答案

袋鼠数学竞赛试题及答案1. 基础计算题:计算下列各题的结果。
- 题目一:\( 56 + 78 - 39 \)- 题目二:\( 48 \times 25 \)- 题目三:\( 3200 ÷ 40 + 76 \)2. 逻辑推理题:小明有5个不同颜色的球,他想从这些球中选出3个来玩。
请问小明有多少种不同的选法?3. 几何题:一个正方形的边长为10厘米,求其周长和面积。
4. 应用题:一家商店出售T恤衫,每件T恤衫的进价是50元,标价是100元。
如果商店决定打8折销售,那么每件T恤衫的利润是多少?5. 数列题:一个等差数列的首项是3,公差是2,求这个数列的第10项。
6. 概率题:一个袋子里有5个红球和3个蓝球,随机抽取一个球,求抽到红球的概率。
7. 组合题:一个班级有30个学生,需要选出5个学生代表班级参加比赛。
如果不考虑顺序,有多少种不同的选法?8. 代数题:解下列方程:\( 3x - 7 = 26 \)9. 统计题:一组数据是:4, 7, 2, 9, 5, 8。
求这组数据的平均数和中位数。
10. 智力题:一个数字去掉第一位是42,去掉最后一位是32,这个数字是什么?答案1. 基础计算题- 题目一:\( 56 + 78 - 39 = 95 \)- 题目二:\( 48 \times 25 = 1200 \)- 题目三:\( 3200 ÷ 40 + 76 = 95 \)2. 逻辑推理题:小明有5个不同颜色的球,选择3个球的选法是\( C(5, 3) = 5! / (3! \times (5-3)!) = 10 \) 种。
3. 几何题:正方形的周长是 \( 4 \times 10 = 40 \) 厘米,面积是\( 10 \times 10 = 100 \) 平方厘米。
4. 应用题:打8折后,T恤衫售价为 \( 100 \times 0.8 = 80 \) 元,利润是 \( 80 - 50 = 30 \) 元。
袋鼠数学国际数学竞赛题

袋鼠数学国际数学竞赛题摘要:一、袋鼠数学竞赛简介1.袋鼠数学竞赛的起源2.竞赛面向的年龄段和级别3.竞赛的宗旨和目标二、袋鼠数学竞赛的特点1.题目趣味性强2.题目涉及多个领域3.鼓励学生用不同方法解题三、袋鼠数学竞赛的题目类型1.选择题2.填空题3.解答题四、袋鼠数学竞赛的评分标准1.正确率2.解题过程3.创意性解题五、参加袋鼠数学竞赛的意义1.提升数学能力2.培养逻辑思维3.激发学习兴趣正文:袋鼠数学国际数学竞赛(Kangaroo Mathematics Competition)是一项在全球范围内举办的青少年数学竞赛,起源于澳大利亚,现在已经发展成为一个国际性的数学竞赛。
该竞赛主要面向小学四年级至高中的学生,根据学生的年龄和年级分为不同级别。
竞赛旨在激发学生对数学的兴趣,提高他们的数学能力,培养他们的逻辑思维和创新能力。
袋鼠数学竞赛的特点在于题目的趣味性强,题目设置不拘泥于传统数学题目,而是涉及到多个领域,如几何、组合、逻辑等。
竞赛鼓励学生用不同的方法解题,注重培养学生的发散性思维。
题目类型包括选择题、填空题和解答题,让学生在各种题型中锻炼自己的数学能力。
袋鼠数学竞赛的评分标准不仅看重学生的正确率,还看重学生的解题过程和创意性解题。
这意味着学生在解题过程中,即使答案不正确,但若能给出有创意的解题思路,也有可能获得一定分数。
这样的评分方式旨在鼓励学生勇于尝试,不怕失败,培养他们独立思考和创新的能力。
参加袋鼠数学竞赛对学生有很多意义。
首先,通过参加竞赛,学生可以提升自己的数学能力,掌握更多数学知识。
其次,竞赛中的题目设置可以培养学生的逻辑思维能力,让他们在面对问题时能更加冷静、理性地分析。
最后,袋鼠数学竞赛的趣味性和挑战性可以激发学生对数学的兴趣,让他们在学习中找到乐趣,为未来的学习打下坚实的基础。
袋鼠竞赛考试题目和答案

袋鼠竞赛考试题目和答案一、单项选择题(每题2分,共20分)1. 袋鼠是澳大利亚特有的动物,以下哪个选项是袋鼠的特征?A. 有翅膀,能飞行B. 有袋,能育幼C. 有尾巴,能游泳D. 有角,能攻击答案:B2. 袋鼠的繁殖季节一般在什么时候?A. 春季B. 夏季C. 秋季D. 冬季答案:B3. 袋鼠的跳跃能力非常强,以下哪个选项是袋鼠跳跃时的动力来源?A. 前肢B. 后肢C. 前肢和后肢D. 尾巴答案:B4. 袋鼠的寿命一般为多少年?A. 5-10年B. 10-15年C. 15-20年D. 20-25年答案:B5. 袋鼠的主食是什么?A. 肉类C. 植物D. 昆虫答案:C6. 袋鼠的耳朵有什么作用?A. 保持平衡B. 散热C. 捕捉声音D. 储存食物答案:B7. 袋鼠的尾巴有什么作用?A. 保持平衡B. 攻击敌人C. 储存食物D. 捕捉声音8. 袋鼠的跳跃速度可以达到多少?A. 10-20公里/小时B. 20-30公里/小时C. 30-40公里/小时D. 40-50公里/小时答案:C9. 袋鼠的跳跃高度可以达到多少?A. 1-2米B. 2-3米C. 3-4米D. 4-5米答案:B10. 袋鼠的跳跃距离可以达到多少?B. 10-15米C. 15-20米D. 20-25米答案:B二、多项选择题(每题3分,共15分)11. 以下哪些是袋鼠的种类?A. 红袋鼠B. 灰袋鼠C. 树袋鼠D. 袋熊答案:ABC12. 以下哪些是袋鼠的天敌?A. 鹰B. 狼C. 狮子D. 袋獾答案:AD13. 以下哪些是袋鼠的繁殖方式?A. 卵生B. 胎生C. 胎生后育幼D. 胎生后独立答案:C14. 以下哪些是袋鼠的生活环境?A. 草原B. 森林C. 沙漠D. 山地答案:ABCD15. 以下哪些是袋鼠的保护措施?A. 建立保护区B. 限制狩猎C. 人工繁殖D. 无为而治答案:ABC三、判断题(每题1分,共10分)16. 袋鼠是澳大利亚的国宝,也是澳大利亚的象征。
袋鼠数学国际数学竞赛题

袋鼠数学国际数学竞赛题在数学领域中,数学竞赛一直是评价学生数学能力的重要方式之一。
而袋鼠数学国际数学竞赛作为一项全球性的数学竞赛,其题目设计独特,涵盖了数学的各个领域,挑战了参赛者的逻辑思维和问题解决能力。
本文将以袋鼠数学国际数学竞赛题为标题,介绍一些典型的数学竞赛题目。
一、立体几何题目袋鼠数学国际数学竞赛中的立体几何题目常常要求考生通过计算、推理和分析来解决问题。
例如,某一题目可能给出一个复杂的立体图形,要求计算其体积或表面积。
这种题目考察了学生对立体几何概念的理解和应用能力。
二、数列与函数题目数列与函数是数学竞赛中常见的题型。
袋鼠数学国际数学竞赛中的数列与函数题目往往要求学生找出规律并推导出数列的通项公式或函数的表达式。
例如,给定一个数列的前几项,要求学生推导出该数列的通项公式,进而计算出指定项的数值。
三、概率与统计题目概率与统计是数学中的一门重要分支,也是袋鼠数学国际数学竞赛中的常见题型。
这类题目通常要求学生计算概率、分析数据、解释图表等。
例如,给定一组数据,要求学生计算出均值、中位数、众数等统计量,并通过数据分析给出相关结论。
四、数论题目数论是数学中的一门独立学科,主要研究整数之间的性质和关系。
袋鼠数学国际数学竞赛中的数论题目常常要求学生通过推理和证明来解决问题。
例如,给定一组整数,要求学生证明其中存在一个或多个整数满足特定条件。
五、代数与方程题目代数与方程是数学中的基础内容,也是袋鼠数学国际数学竞赛中的常见题型。
这类题目通常要求学生解方程、化简表达式、求解未知数等。
例如,给定一个方程,要求学生求解出方程中的未知数,并给出解的范围。
六、几何推理题目几何推理是袋鼠数学国际数学竞赛中的一类重要题目。
这类题目常常要求学生通过观察图形、分析规律来进行推理和判断。
例如,给出一组图形,要求学生找到其中的规律,并根据规律选择正确的图形作为答案。
通过以上几个典型的数学竞赛题目,我们可以看到袋鼠数学国际数学竞赛的题目设计非常灵活多样,旨在考察学生的数学思维能力和解决问题的能力。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
Back to All Problems Page
MATH KANGAROO 2004 in USA
Level of Grades 3 - 4
3 points each
1. 2001+ 2002 + 2003 + 2004 + 2005 =
A) 1,015 B) 5,010 C) 10,150 D) 11,005 E) 10,015
2. Marek was 4 years old when his sister was born. Today he blew out all 9 candles on his birthday cake. What is the difference between Marek’s and his sister’s age today?
A) 4 years B) 5 years C) 9 years D) 13 years E) 14 years
3. The picture below shows a road from town A to town B (indicated by solid line) and a detour (marked by a dash line) caused by renovation of the section CD. How many kilometres longer is the road from town A to town B because of the detour now?
A) 3 km B) 5 km C) 6 km D) 10 km E) This cannot be calculated.
4. Which of the results below is not identical to the difference 671 – 389?
A) 771 – 489 B) 681 – 399 C) 669 – 391 D) 1871 – 1589 E) 600 –318
5. There were some birds sitting on the telegraph wire. At one moment, 5 of them flied away and after some time, 3 birds came back. At that time there were 12 birds sitting on the wire. How many birds were there at the very beginning?
A) 8 B) 9 C) 10 D) 12 E) 14
6. Which numbers are inside a rectangle and inside a circle but not inside a triangle at the same time?
A) 5 and 11 B) 1 and 10 C) 13 D) 3 and 9 E)
6, 7 and 4
7. Buildings on Color Street are numbered from 1 to 5 (see the picture).
Each building is colored with one of the following colors: blue, red,
yellow, pink, and green. It is known that:
– The red building neighbours with the blue one only.
– The blue building is between the red one and the green one.
What is the color of the building numbered with 3?
A) Blue B) Red C) Yellow D) Pink E) Green
8. How many white squares need to be shaded so that the number of
shaded squares equals exactly to half of the number of white squares?
A) 2 B) 3 C) 4 D) 6 E) It is
impossible to calculate it.
4 points each
9. Five identical sheets of a plastic rectangles were divided
into white and black squares. Which of the sheets from A to E
has to be covered with the sheet to the right in order to get
totally black rectangle?
A: B: C: D:E:
10. The scales in the pictures had been balanced. There are pencils and a pen on the arms of the scales. What is the weight of the pen in grams?
A) 6 g B) 7 g C) 8 g D) 9 g E) 10 g
11. I notice four clocks on the wall (see the picture). Only one of them shows correct time. One of them is 20 minutes ahead, another is 20 minutes late, and the other is stopped. What is the time at the moment?
among them. There are 14 students on Mathew’s left, and Maria is among them. There are 7 students between Maria and Mathew. How many students are in this class?
A) 37 B) 30 C) 23 D) 22 E) 16
20. The sum of the digits of the 10-digit number is 9.What is the product of the digits of this number?
A) 0 B) 1 C) 45 D) 9 x 8 x 7 x…..x 2 x 1
E) 10
21. Out of 125 small, white and black cubes, the big cube was formed (see the picture). Every two
adjacent cubes have different colors. The vertices of the big cube are black. How many white cubes
does the big cube contain?
A) 62 B) 63 C) 64 D) 65 E) 68
22. A lottery-ticket was 4 dollars. Three boys: Paul, Peter, and Robert made a contribiution and bought two tickets. Paul gave 1 dollar, Peter gave 3 dollars, and Robert gave 4 dollars. One of the tickets they bought was worth 1000 dollars. Boys shared the award fairly, meaning, proportionally to their contributions. How much did Peter receive?
A) 300 B) 375 C) 250 D) 750 E) 425
23. In three soccer games the Dziobak’s team scored three goals and lost one. For every game won the team gets 3 points, for
a tie it gets 1 point, and for the game lost it gets 0 points. For sure, the number of points the team earned in those three games was not equal to which of the following numbers?
A) 7 B) 6 C) 5 D) 4 E) 3
24. In every white section of a diagram, the products of two numbers from grey sections – one from
above and one from the left – was placed (for example: 42 = 7 • 6 ). Some of these products are
represented by letters. Which two letters represent the same number?
A) L and M B) T and N C) R and P D) K and P E) M and S
back to all problems page。
