Duality and other Exotic Gauge Dynamics in Softly Broken Supersymmetric QCD
2022款福特 Bronco 两门和四门车型规格说明书

* H orsepower and torque ratings based on premium fuel per SAE J1349 standard. Horsepower and torque are independent attributes and may not be achieved simultaneously.For editorial use only. Information correct at time of publication. Check for updates.BODYVehicle type Midsize two-door and four-door rugged 4x4 SUVTrim series Base, Big Bend ™, Black Diamond ™, Everglades ™, Outer Banks ™, Badlands ™, Wildtrak ™, Raptor ™ (see specs on separate page)Option packages Mid, High, Lux, Sasquatch ™ Package Final assembly locationMichigan Assembly Plant, Wayne, MichiganENGINES2.3-liter EcoBoost ® 2.7-liter EcoBoost Configuration Ti-VCT GTDI I-4Ti-VCT GTDI 60-degree V6Displacement 138 cu. in. (2,264 cc)164 cu. in. (2,694 cc)Bore x stroke 3.45 in. x 3.7 in. (87.55 mm x 94 mm) 3.27 in. x 3.27 in. (83 mm x 83 mm)Compression ratio 10.0:110.0:1Fuel deliveryDirect injectionPort fuel/direct injectionEngine control system Chassis integrated powertrain control module Chassis integrated powertrain control module Engine block material High-pressure die cast aluminum alloyCompacted graphite ironPistonsLightweight, high-strength cast aluminum with low-friction skirt coating and steel ring Lightweight, high-strength cast aluminum with low-friction skirt coating and steel ring Connecting rods Forged steel Forged steel Crankshaft Forged steelForged steelValve diameter/lift Intake, 32.5 mm/8.9 mm Exhaust, 30 mm/7.8 mm Intake, 32.50 mm/10 mm Exhaust, 28.30 mm/9.0 mmValve lifters Direct acting mechanical bucket Roller finger follower, hydraulic compensating lash adjusters Intake Composite, split plenum Composite Ignition Coil on plugCoil on plugInduction Twin-scroll turbocharger with electrically actuated wastegate Dual mono-scroll turbochargers with electrically actuated wastegate ExhaustCast ironIntegrated cylinder head casting Recommended fuel Regular unleaded 87 octane minimum Regular unleaded 87 octane minimum Oil capacity 6.2 quarts 7.0 quarts Coolant capacity 11.1 liters11.7 litersExhaust system Single catalyst with resonator and single muffler Double catalyst with resonator and single muffler Alternator Standard: Single 240A Standard: Single 240A Battery groupH7 AGM 80 AH 800 CCA H7 AGM 80 AH 800 CCA SAE horsepower (regular fuel)275 @ 5,700 rpm 315 @ 5,500 rpm SAE torque (regular fuel)315 @ 3,400 rpm 410 @ 3,250 rpm SAE horsepower (premium fuel)*300 @ 5,700 rpm 330 @ 5,250 rpm SAE torque (premium fuel)*325 @ 3,400 rpm 415 @ 3,100 rpm Build locationCleveland Engine PlantLima Engine Plant* M aximum ratios with high-capability option packages.For editorial use only. Information correct at time of publication. Check for updates.DRIVETRAINLayoutLongitudinally mounted front engine with center-mounted transfer case, independent front axles/solid rear axlesTRANSMISSIONSStandard 7-speed (6+1 crawler gear) Getrag manual (offered on 2.3-liter engine only)Optional10-speed automatic (available on both 2.3- and 2.7-liter engines)Gear Ratios7-Speed Manual 10-Speed Automatic Crawler 6.588:1First 4.714:1First 4.283:1Second 2.997:1Second 2.365:1Third 2.149:1Third1.453:1Fourth 1.769:1Fourth 1.000:1Fifth 1.521:1Fifth 0.776:1Sixth 1.275:1Sixth 0.646:1Seventh 1.000:1Reverse-5.625:1Eight 0.853:1Ninth 0.689:1Tenth 0.636:1Reverse-4.885:1Transfer Cases StandardOptional4x4 with part-time engagement electronic shift-on-the-fly, 2.72:1 low ratioAdvanced 4x4 with 4A mode automatic on-demand engagement, 3.06:1 low ratioMaximum crawl ratios*79.92:1 – 7-speed manual with standard ESOF57.19:1 – 10-speed speed automatic with standard ESOF 94.75:1 – 7-speed manual with optional Advanced 4x467.80:1 – 10-speed automatic with optional Advanced 4x4Flat-tow capability Yes Yes Axles FrontRearStandard Dana ™ AdvanTEK ® M190 independent front suspension Dana 44™ AdvanTEK M220 solid rear differential OptionalDana ™ AdvanTEK ® M210 independent front suspension with Spicer ® Performa-TraK electronic lockerDana 44™ AdvanTEK M220 solid rear differential with Spicer ®Performa-TraK electronic lockerCHASSISTypeBody-on-frame; fully boxed high-strength steel frame with seven cross members, High-Performance Off-Road Stability Suspension (HOSS) System with low-mass independent front suspension, five-link solid rear axle with Panhard rod and outboard coilover springs Suspensions FrontRearStandard Twin forged alloy A-arms with long-travel coil-over springs, HOSS-tuned heavy-duty dampers 220 mm solid rear axle with long-travel, variable rate coil-over springs, HOSS-tuned heavy-duty dampers OptionalBilstein ® position-sensitive dampers with Bilstein position-sensitive dampers with* R equires heavy-duty modular front bumper.For editorial use only. Information correct at time of publication. Check for updates.BODYTwo-door Four-door Tops standard(varies by trim series)Removable hardtop Removable soft-top Removable hardtopRoof panel weight (pounds)14.0 front left, 15.4 front right 13.6 front left, 14.5 front right, 28.1 mid panel Doors/storage Two removeable frameless doors Four removable frameless doors Available rear storage for four doors Door weight (pounds)Front 62Front 55/Rear 44Modular section Doors, roof panels, front fenders, grille, fender flares, rear quarter panels, bumpersRock rail Standard on Black Diamond, Everglades and Badlands series dealer installed accessory on all other Bronco series Tube stepsStandard on Outer Banks, available on Big Bend and WildtrakUnderbody protectionBlack Diamond, Everglades and Widltrak (optional): Five steel underbody shields including engine shield, transfer case shield, fuel tank shield, shin guards and heavy-duty front bash plate*Badlands: Six steel underbody shields including engine shield, transfer case shield, fuel tank shield, shin guards, stabilizer-bar shield and heavy-duty front bash plate*Modular bumpers Heavy-duty powder-coated modular steel front bumper with heavy-duty bash plate standard on Everglades, available on all other seriesWashout vinyl flooring with drain plugsStandard on Black Diamond, Everglades and Badlands series Trail sights with 150-pound tie-down capacityStandard on all Bronco seriesBRAKESFront RearType Four-wheel power disc brakes with four-sensor, four-channel antilock braking system and electronic stability control Boost2.3-liter engine, vacuum brake boost, 2.7-liter engine, electronic brake boost Brake configuration Vented discs, twin-piston floating caliper Discs with single-piston floating caliper, integral electronic parking brake Rotor diameter/thickness 311 mm x 34 mm 308 mm x 24 mm Pad material Non-asbestos organic Non-asbestos organic Caliper type Twin 51-mm floating Single 54-mm floating Pad swept area413 cm 2373 cm2TypeThree-mode rack-and-pinion steering with standard, comfort and sport modes controlled via Terrain Management System ™ with G.O.A.T. Modes ™ (Goes Over Any Type of Terrain)Turning radius (curb-to-curb)35.53 feet (10.83 m)12.13 m (Badlands)/12.15 m (Sasquatch)BANG & OLUFSEN ©2021 and B&O ©2021. BANG & OLUFSEN ™ and B&O ™ are registered trademarks of Bang & Olufsen Group. Licensed by Harman Becker Automotive Systems Manufacturing Kft. All rights reserved.For editorial use only. Information correct at time of publication. Check for updates.TECHNOLOGYTerrain Management with G.O.A.T. ModesStandard modes, Base (5): Normal, Eco, Sport, Slippery and SandBig Bend, Outer Banks (6): Normal, Eco, Sport, Slippery, Mud/Ruts and SandBlack Diamond and Everglades (7): Normal, Eco, Sport, Slippery, Mud/Ruts, Sand and Rock Crawl Badlands, First Edition (7): Normal, Eco, Slippery, Mud/Ruts, Baja, Sand and Rock Crawl Wildtrak (7): Normal, Eco, Sport, Slippery, Baja, Mud/Ruts and Sand Trail control Standard on all Bronco series with 10-speed automatic transmission Trail turn assist Standard on all Bronco series with 10-speed automatic transmission Trail one-pedal driveStandard on 2.7-liter V6 models with 10-speed automatic transmissionBaseBig Bend Black Diamond Everglades Outer Banks Badlands Wildtrak HMI/audio system, SYNC ® 4 8-inch8-inch 8-inch 12-inch 8-inch 8-inch 8-inchABS/stability control electric power-assisted steering, and ABSAirbagsFirst-row driver and passenger dual-stage front and seat side airbagsAll rows: Safety Canopy side-curtain airbags with rollover sensors for all rows*D enotes Sasquatch ™ PackageFor editorial use only. Information correct at time of publication. Check for updates.WHEELSSeries StandardBase 16-inch bright polished silver-painted steel Standard Big Bend 17-inch Carbonized Gray-painted aluminum Standard Black Diamond 17-inch black gloss-painted black steel Optional Black Diamond 17-inch black mid-gloss-painted aluminum Standard Everglades 17-inch Carbonized Gray-painted alloy wheelsStandard Outer Banks 18-inch bright machined black high-gloss-painted aluminum Standard Badlands 17-inch machined Carbonized Gray-painted aluminumOptional Badlands 17-inch black high-gloss-painted aluminum with Carbonized Gray beauty ring, beadlock-capable StandardWildtrak*17-inch black high-gloss-painted aluminum with black beauty ring, beadlock-capableSasquatch Package SeriesOptionalBase, Big Bend, Black Diamond, Badlands17-inch black high-gloss-painted aluminum with warm alloy beauty ring, beadlock-capableLIGHTING/CONVENIENCEBaseBig Bend Black Diamond Everglades Outer Banks Badlands Wildtrak Headlamps Auto on/off LED Auto high-beamS S S S S S S Bronco signature LED lighting N/A O N/A N/A S S S LED fog lampsN/A S S S S S S Mirror LED approach lamps and LED spotlightsN/A N/A N/A N/A O O O Overhead upfitter switches with six-pre-wired user-defined electrical connectionsO O S S O S S Dual-control HVAC automatic temperature control N/A O O S S O S Heated front seatsN/A O O S S O S Central locking system with keyless entry and push-button start S S S S S S S Push button startS S S S S S S Intelligent access (lock/unlock)N/A O O S S O S Remote start system with FordPass Connect (automatic transmission models only)S S S S S S S Power window SSSSSSSAccessory ElectricalOne 110V AC 400 watt power outlet (Mid Package and up; rear of center console)Power points (12V) – one (1) center floor console, one (1) cargo areaStandard: Smart Charging Multimedia USB Ports, First Row – One (1) USB-A and one (1) USB-C; Smart Charging USB Ports, Second Row – One (1) USB-A and one (1) USB-C in the back side of the center floor consoleOptional: Lux package – Smart Charging USB Ports, dash board – One (1) USB-A and one (1) USB-CDIMENSIONS/CAPACITIES (INCHES)Two-door Four-doorWheelbaseAll series100.4116.1LengthBase173.7189.4Big Bend173.7189.4Wildtrak173.7189.5Badlands174.8190.5Everglades N/A198.9HeightBase71.973.0 (soft-top)Big Bend72.972.9Wildtrak75.275.3Badlands73.873.9( W hen available roof racks are installed, add 3.6 inches to two-doormodels, 3.4 inches to four-door models for maximum height)Everglades N/A78.7 (includes standard roof rack) Width (with mirrors folded)Base/Big Bend75.975.9Wildtrak79.379.3Badlands76.376.3Everglades N/A79.4 (includes mirrors)Track width, front/rearBase/Big Bend/Badlands65.0/65.065.0/65.0Wildtrak/Everglades66.9/66.966.9/66.9Seating capacity Four FivePassenger volume, hardtop (cu. ft.)99103.7Passenger volume, soft-top (cu. ft.)N/A108.2Behind first row, hardtop (cu. ft.)52.377.6Behind first row, soft-top (cu. ft.)N/A83.0Behind second row, hardtop (cu. ft.)22.435.6Behind second row, soft-top (cu. ft.)N/A38.3HeadroomFirst row, hardtop41.040.8First row, soft-top N/A43.3Second row, hardtop39.840.1Second row, soft-top N/A41.1LegroomFirst row43.143.1Second row35.736.3Hip roomFirst row56.355.9Second row43.354.8Shoulder roomFirst row57.157.1Second row51.856.5Fuel capacity (gallons)16.920.8For editorial use only. Information correct at time of publication. Check for updates.* R emote start on automatic transmission models only. **Trailer tow capability determined using SAE J2807 standard. † Requires optional Ford Accessories hitch. †Additional options may decrease payload.For editorial use only. Information correct at time of publication. Check for updates.OPTION GROUP HIGHLIGHTSMid Package equipment group highlightsSYNC 4 with 8-inch LCD touch screen/audio system, connected navigation, dual-zone HVAC, front-rowheated seats, two-door intelligent access, remote start system,* Ford Co-Pilot360, reverse sensing system, rear passenger power outletHigh Package equipment group highlightsIncludes all Mid Package content plus:SYNC 4 with 12-inch LCD touch screen/audio system and information on-demand panel, 360-degree camera, forward sensing system, additional sound deadening, mirror-mounted LED approach lamps and spotlights Lux Package equipment group highlightsIncludes all Mid and High Package content plus:10-speaker B&O Sound System by Bang & Olufsen, adaptive cruise control and evasive steering assist, heated steering wheel, connected built-in navigation with three years of service, wireless charging padand dash-mounted USB-A and USB-C smart charging portsBase 2.3-liter EcoBoost I-47-speed manual 2,3384,2941,2465,5402,9003,0003,500†8,780Big Bend 2.3-literEcoBoost I-47-speed manual 2,3444,3041,2365,5402,9003,0003,5008,780Black Diamond 2.3-liter EcoBoost I-47-speed manual 2,4904,5871,0535,6402,9003,0003,5008,780Badlands 2.3-liter EcoBoost I-47-speed manual 2,5764,6991,0215,7202,9003,0003,5008,780Base 2.3-liter EcoBoost I-410-speed SelectShift automatic 2,3534,3141,2265,5402,9003,0003,500†8,480Big Bend 2.3-liter EcoBoost I-410-speed SelectShift automatic 2,3594,3241,2165,5402,9003,0003,5008,780Black Diamond 2.3-liter EcoBoost I-410-speed SelectShift automatic 2,5044,6051,0345,6402,9003,0003,5008,480Outer Banks 2.3-liter EcoBoost I-410-speed SelectShift automatic 2,4024,4131,1275,5402,9003,0003,5008,480Badlands 2.3-liter EcoBoost I-410-speed SelectShift automatic 2,5914,7191,0015,7203,0003,0003,5008,780Base 2.7-liter EcoBoost V610-speed SelectShift automatic 2,4934,4661,2345,7003,0003,0003,500†8,740Big Bend 2.7-liter EcoBoost V610-speed SelectShift automatic 2,4994,4761,2245,7003,0003,0003,5008,740Black Diamond 2.7-liter EcoBoost V610-speed SelectShift automatic 2,6444,7571,0225,7803,0003,0003,5008,840Outer Banks 2.7-liter EcoBoost V610-speed SelectShift automatic 2,5424,5651,1355,7003,0003,0003,5008,740Badlands 2.7-liter EcoBoost V610-speed SelectShift automatic 2,7314,8719895,8603,0003,0003,5008,840Wildtrak2.7-liter EcoBoost V610-speed SelectShift automatic2,5444,5741,0695,7403,0003,0003,5008,740†Requires optional Ford Accessories hitch.For editorial use only. Information correct at time of publication. Check for updates.WARRANTYBumper-to-bumper Three years/36,000 miles Powertrain Five years/60,000 miles CorrosionFive years/unlimited miles Roadside assistance24-hour/day (3 years/36 miles)。
AdsCft在凝聚态中应用简介
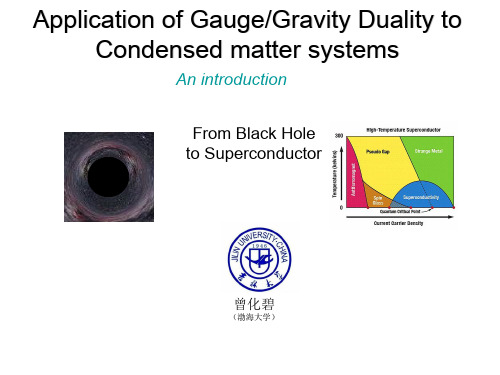
Phys.Rev.Lett. 94 (2005) 111601…
• Holographic Universe Paul McFadden, Kostas Skenderis, The
•
Far-from equilibruim statistics.
(Tuburlance, superfluid/superconductor dynamics,non-equilibruim phase transition...)
•
AdS/CMT have already covered many fields in CMT.
Holographic Universe, arXiv:1001.2007.
• AdS/CM (Condensed Matter). CM/Gravity? (The Concept of Emergent in CM)
Supersymmetry emerged from a 2+1 dimension lattice model arXiv:1009.5127. A review from condensed matter theorist: Subir Sachdev, Condensed matter and AdS/CFT, arXiv:1002.2947. A review from General Relativity theorist: Gary T. Horowitz, Surprising Connections Between General Relativity and Condensed Matter, arXiv:1010.2784.
26个英文字母书写方式

26个英文字母书写方式The 26 letters of the English alphabet have been in use for centuries, serving as the fundamental building blocks of the written language. These letters, each with its unique shape and sound, have evolved over time, reflecting the rich cultural and linguistic histories of the regions where they have been employed.The origins of the modern English alphabet can be traced back to the ancient Phoenician script, which was adopted by the Greeks and later by the Romans. The Roman alphabet, in turn, became the basis for the Latin alphabet that is used in English and many other European languages.The first letters of the English alphabet, A through Z, are derived from the corresponding letters in the Latin alphabet. Each letter has a distinct name and sound, and they can be combined in various ways to form words, sentences, and entire written works.The letter A, for example, is one of the most commonly used letters in the English language. Its name is derived from the Greek letter alpha, and it represents the first sound in the alphabet. The shape of the letter A has evolved over time, from the angular forms used inancient scripts to the more rounded and symmetrical shapes we recognize today.Similarly, the letter B has its origins in the Phoenician letter beth, and it represents the second sound in the alphabet. The shape of the letter B has also undergone changes, from the more angular forms used in early Latin scripts to the more rounded and consistent shapes we see in modern typography.The letter C, derived from the Greek letter gamma, represents the third sound in the alphabet and is one of the most versatile letters in the English language. It can be used to represent the hard "k" sound, as in "cat," or the soft "s" sound, as in "city."The letter D, derived from the Phoenician letter daleth, represents the fourth sound in the alphabet and is often used to convey a sense of strength, authority, or finality in words and names.The letter E, derived from the Greek letter epsilon, is the most common letter in the English language and is essential for the formation of many words and grammatical structures. Its shape has evolved from the more angular forms used in early Latin scripts to the more rounded and consistent shapes we see today.The letter F, derived from the Phoenician letter waw, represents thesixth sound in the alphabet and is often used to convey a sense of force, motion, or emphasis in words and phrases.The letter G, derived from the Greek letter gamma, represents the seventh sound in the alphabet and is known for its versatility, as it can be used to represent both the "g" and "j" sounds in English.The letter H, derived from the Phoenician letter heth, represents the eighth sound in the alphabet and is often used to convey a sense of aspiration or breath in words and phrases.The letter I, derived from the Greek letter iota, represents the ninth sound in the alphabet and is known for its simplicity and elegance in written form.The letter J, derived from the Latin letter Iota, represents the tenth sound in the alphabet and is often used to convey a sense of playfulness, exoticism, or uniqueness in words and names.The letter K, derived from the Greek letter kappa, represents the eleventh sound in the alphabet and is often used to convey a sense of power, strength, or authority in words and phrases.The letter L, derived from the Phoenician letter lamed, represents the twelfth sound in the alphabet and is known for its graceful, flowingshape in written form.The letter M, derived from the Phoenician letter mem, represents the thirteenth sound in the alphabet and is often used to convey a sense of solidity, stability, or permanence in words and phrases.The letter N, derived from the Phoenician letter nun, represents the fourteenth sound in the alphabet and is known for its versatility, as it can be used to represent both the "n" and "ng" sounds in English.The letter O, derived from the Greek letter omicron, represents the fifteenth sound in the alphabet and is known for its simple, elegant shape in written form.The letter P, derived from the Phoenician letter pe, represents the sixteenth sound in the alphabet and is often used to convey a sense of power, precision, or progress in words and phrases.The letter Q, derived from the Phoenician letter qoph, represents the seventeenth sound in the alphabet and is often used to convey a sense of mystery, intrigue, or uniqueness in words and names.The letter R, derived from the Phoenician letter resh, represents the eighteenth sound in the alphabet and is known for its versatility, as it can be used to convey a sense of motion, energy, or power in wordsand phrases.The letter S, derived from the Phoenician letter shin, represents the nineteenth sound in the alphabet and is known for its versatility, as it can be used to represent both the "s" and "z" sounds in English.The letter T, derived from the Phoenician letter taw, represents the twentieth sound in the alphabet and is often used to convey a sense of finality, authority, or precision in words and phrases.The letter U, derived from the Greek letter upsilon, represents the twenty-first sound in the alphabet and is known for its simple, elegant shape in written form.The letter V, derived from the Latin letter V, represents the twenty-second sound in the alphabet and is often used to convey a sense of strength, power, or dynamic movement in words and phrases.The letter W, derived from the Phoenician letter waw, represents the twenty-third sound in the alphabet and is known for its unique shape, which is often used to convey a sense of duality or complexity in words and names.The letter X, derived from the Greek letter chi, represents the twenty-fourth sound in the alphabet and is often used to convey a sense ofmystery, complexity, or hidden meaning in words and phrases.The letter Y, derived from the Greek letter upsilon, represents the twenty-fifth sound in the alphabet and is known for its versatility, as it can be used to represent both the "y" and "i" sounds in English.Finally, the letter Z, derived from the Greek letter zeta, represents the twenty-sixth and final sound in the alphabet. It is often used to convey a sense of energy, dynamism, or uniqueness in words and names.Together, these 26 letters form the foundation of the English language, allowing us to express ourselves through written communication. From the simple and elegant forms of the A and O to the more complex and dynamic shapes of the X and Z, each letter has its own unique character and history, contributing to the rich tapestry of the written word.。
宇宙ppt课件英文

Black Hole Types
Different types of black holes including stellar mass black holes, supermassive black holes, and intermediate mass black holes and their characteristics and distribution in the universe.
宇宙ppt课件英文
CATALOGUE
目录
IntroductionThe Structure of the UniverseThe Evolution of the UniverseThe Science of the UniverseThe Applications of the UniverseConclusion
The evolution of stars over time, including the life cycle of stars from birth to death and the different stages of stellar evolution.
Planet Formation
Quasar Properties
The properties of quasars including their luminosity, redshift, and spectral energy distribution.
糖果名字的寓意作文英语

Growing up, I was always fascinated by the world of sweets, and it wasnt just the taste that drew me in, but also the names of the candies that seemed to hold a story of their own. Each name was like a small window into a world of imagination and creativity, and it was this curiosity that led me on a journey to understand the deeper meanings behind these sugary delights.One of the first candies that caught my attention was Jujube. The name itself sounded exotic and mysterious, and as I learned more about it, I discovered that it was derived from the Chinese word for the datelike fruit it resembled. The jujube is not just a candy but also a symbol of longevity and good health in Chinese culture. This realization made me appreciate the candy even more, as it was not only a treat for the palate but also a carrier of cultural significance.Another candy that intrigued me was Smarties. The name, with its connotation of intelligence, was a clever marketing strategy that made the candy feel like more than just a simple sweet. It was as if eating a Smartie could somehow make you smarter, or at least give you a momentary boost of confidence. This was a great example of how a candys name could influence its perception and appeal to consumers.The Mars Bar was another interesting case. Named after the founders family name, it was a testament to the personal connection that can exist between a product and its creator. The name also evoked images of the planet Mars, adding a sense of adventure and exploration to the candy. It was as if each bite of the Mars Bar was a step into the unknown, a journeyto a new world of taste and texture.The Twix was a candy that played with the idea of duality. The name, a combination of twin sticks, suggested a sense of partnership and togetherness. It was a candy that was meant to be shared, a symbol of unity and companionship. This idea of sharing and togetherness was a powerful message that resonated with many people, making the Twix a popular choice for those looking to enjoy a sweet treat with a friend or loved one.The Snickers was a candy that had a playful and mischievous feel to it. Named after the Mars familys favorite horse, the name evoked images of a spirited and energetic animal. This playful spirit was reflected in the candy itself, with its combination of chocolate, caramel, and peanuts creating a dynamic and lively taste experience.The Kit Kat was a candy that was all about taking a break. The name was a play on the phrase give me a break, suggesting that enjoying a Kit Kat was a momentary escape from the stresses and pressures of everyday life. This idea of taking a break was a powerful message that resonated with many people, making the Kit Kat a popular choice for those looking to enjoy a moment of relaxation and indulgence.In conclusion, the names of candies are more than just labels they are stories, symbols, and messages that add depth and meaning to these sweet treats. From the cultural significance of the Jujube to the playful spirit of the Snickers, each candys name tells a unique story and conveys adistinct message. As a lover of sweets, I find this exploration of candy names to be a delicious journey of discovery and appreciation.。
动物园建筑

[ UeD ] 099 | 02 | 2016设计一座动物园建筑并非易事。
建筑师是应重现动物在自然中的栖息所,还是提出一个抽象或者现代主义的宣言?伦敦动物园企鹅池撼人心魄的混凝土双螺旋坡道属于后者。
或者能否参考动物居住者身上的一些表征,形成诸如逶迤的蛇舍和硕大的象笼之类的设计?区分“人类居住的建筑”和“动物居住的建筑”真的能够解决问题吗?“人类”的建筑有专门的历史,有自己的传统与风格—古典主义、功能主义、地域主义、现代主义和反构成主义等,不一而足。
而动物园建筑呢?动物们在大自然中的住所如何与人类的相比?对建筑师而言,仅仅去仿制掏空的树洞、岩穴、树枝编成的巢窠,还有蜂窝的几何形状以及螺壳完美的螺旋纹就够了吗?显而易见,动物建筑并不是房屋那么简单。
概念。
生活在21世纪之初的我们能否换一种方式思考动物园建筑?比如超越“人类”建筑与“动物”建筑的二分法,并为二者建立共同的语汇?鸟舍除了做鸟儿们的屋舍,能否也将游客容纳其中?那些通常用来庇护狮子、犀牛和长颈鹿的原木、木梁和树干结构是否同样也能遮蔽游客餐厅设施?毕竟,这里的动物们和人类一样,都已经在巴黎生息繁衍了几代之久。
二重性。
因此我们所构想的是一种二重建筑的形式造型,或者说一种具备二重性的建筑。
其新颖处在于兼顾文化与自然、人类与动物、技术性与异域风情的形式。
游客入口处就像一座巨型鸟舍,有经年生长的繁密草木环绕。
一些相对小型的建筑—有些称作鸟舍,有些称作凉亭—既可供狨猴栖息,又能接纳来此游览的学生们。
每一处都经过了深思熟虑,每个细节都有充分的理由,整个设计没有丝毫的松弛大意。
堆叠起来的数米长的木梁巧妙地包裹住了人与动物设施的肋骨状金属板。
建筑于是有着双层表皮—外层融于景观,内层则保证了动物园的日常运行。
表皮。
没错就是这个词。
21世纪的建筑师们已经不再把立面、檐口和屋顶挂在嘴边,而是谈论“表皮”与“外部环境”。
不同于房屋和屋顶,表皮并无先决的造型—它可以是任何造型,甚至根本没有造型。
《格列佛游记》英文读后感

《格列佛游记》英文读后感Gulliver's Travels: A Journey of Observations and Reflections"Travel broadens the mind", a quote that is often heard and widely accepted. Yet, Gulliver's Travels by Jonathan Swift takes this notion to a whole new level. The novel, which was first published in 1726, remains one of the most popular works of English literature, largely thanks to the timeless themes it explores.The novel tells the story of Lemuel Gulliver, a ship's surgeon who sets sail on an adventure in the South Seas. He encounters strange lands and peculiar creatures along the way, from Lilliputians who are only six inches tall, to giants who are 60 feet tall. Through his travels, Gulliver observes the customs, beliefs, and practices of these different societies, and reflects on the human condition in general.One of the most striking features of Gulliver's Travels is its satirical nature. Swift uses the novel as a vehicle to voice his criticisms of contemporary society and politics. For instance, the Lilliputian society is beset by absurd rules and regulations that serve no practical purpose, but are fiercely enforced nonetheless. Meanwhile, the Brobdingnagians, who appear at first to be idyllic and benign, are revealed to be just as flawed and cruel as any other society when Gulliver observes their treatment of their prisoners. Swift's satire is not limited to his portrayal of fantastical societies. He also takes aim at the flaws and foibles of humanity more generally. One particularly powerful moment occurs when Gulliver reflects on his own "civilized" society and the atrocities it hascommitted in the name of progress and colonialism. He notes that, despite its advanced technology and culture, his own society lacks the basic humanity and compassion that he has observed in some of the societies he has encountered on his travels.At its heart, Gulliver's Travels is a novel about the quest for knowledge and understanding, and the dangers and pitfalls that come along with it. Gulliver's journey takes him to the ends of the earth and beyond, but with each stop he finds that he is still no closer to understanding the mysteries of the world. It is a humbling reminder that, no matter how much we learn or how far we travel, there will always be more to discover and more to learn.In conclusion, Gulliver's Travels is a timeless masterpiece of literature that has much to offer readers of all ages and interests. Its fantastical settings and creatures are irresistible to the imagination, but it is the novel's deeper themes of satire, humanity, and the quest for knowledge that make it truly unforgettable. Swift's work is a testament to the power of literature to inspire reflection and introspection, and to the enduring relevance of the questions it asks.In addition to its exploration of societal flaws and the quest for knowledge, Gulliver's Travels also delves into themes such as power, control, and morality. Swift highlights the dangers of unchecked power and the corrupting influence it can have on individuals and societies. This is most evident in the portrayal of the Houyhnhnms, a race of intelligent horses who have managed to create a utopian society based on reason and logic. However, as Gulliver discovers, even the seemingly perfect Houyhnhnms are not immune to the allure of power and the temptation to exert control over others.The novel also grapples with questions of morality, particularly in its depiction of the Yahoos, a brutish and uncivilized race that represents humanity at its most base and animalistic. Gulliver is forced to confront the uncomfortable truth that, while he may be intellectually superior to the Yahoos, he is not necessarily morally superior to them. This realization leads him to question the very foundations of his own society and the values upon which it is built. One of the key strengths of Gulliver's Travels is its ability to engage readers on multiple levels. On one hand, it is a thrilling adventure story that takes readers to exotic locations and introduces them to fascinating new cultures. On the other hand, itis a work of incisive social commentary that exposes the flaws and failings of the world in which we live. This duality of purpose means that the novel can be appreciated by readers of all ages and backgrounds, and its enduring popularity is a testament to its universal appeal.Another aspect of the novel that remains relevant today is its exploration of the intersection between science and society. Gulliver's travels take him to lands where the inhabitants have developed advanced technologies or are well-versed in scientific concepts. However, Swift uses these settings to highlight the dangers of unbridled scientific advancement and the ways in which scientific progress can be abused in the pursuit of power and control. This is a theme that continues to resonate in contemporary society, as debates rage over issues such as genetic engineering, artificial intelligence, and climate change.Ultimately, Gulliver's Travels is a work of timeless significance that continues to capture the imaginations of readers around the world. Through its satire, themes, and characters, the novel offers a powerful commentary on the world in which we live and the issues that continue to shape our lives. It is a reminder that, while society may change over time, the fundamental questions and concerns that we grapple with as individuals and as a species remain largely the same.。
英语作文模板中国元素

英语作文模板中国元素Chinese Elements in English Writing。
Chinese elements have been widely integrated into English writing, adding unique cultural charm to the literary works. From traditional Chinese symbols to modern cultural phenomena, the influence of Chinese elements on English writing is profound and diverse.First and foremost, traditional Chinese symbols, such as the dragon, the phoenix, and the panda, have been frequently used in English writing to represent Chinese culture. These symbols are often employed to convey a sense of mystery, power, and grace, which are deeply rooted in Chinese mythology and folklore. For example, the dragon is a symbol of strength and good luck in Chinese culture, and it has been featured in numerous English literary works as a powerful and mythical creature. Similarly, the panda, as a national treasure of China, has also become a popular symbol in English writing, representing peace, harmony, and cuteness. By incorporating these traditional Chinese symbols, English writers are able to infuse their works with a touch of exoticism and cultural richness.In addition to traditional symbols, Chinese philosophy and wisdom have also made a significant impact on English writing. The teachings of Confucius, Laozi, and other Chinese philosophers have inspired many English writers to explore the themes of morality, harmony, and the pursuit of wisdom. For instance, the concept of yin and yang, which embodies the duality and balance of the universe in Chinese philosophy, has been adopted by English writers to illustrate the complexity and interconnectedness of human nature and the world. Moreover, the idea of "wu wei" (non-action) from Daoism has been incorporated into English literature to convey the notion of naturalness and spontaneity. By drawing on these profound philosophical ideas, English writers are able to create thought-provoking and introspective works that resonate with readers across different cultural backgrounds.Furthermore, the rich cultural heritage of China, including its traditional festivals, customs, and rituals, has also found its way into English writing. The celebration of Chinese New Year, the reverence for ancestors, and the practice of tea ceremony are just a few examples of Chinese cultural elements that have been portrayed in English literary works. By depicting these cultural practices, English writers are able to offer readers a glimpse into the vibrant and diverse traditions of China, fostering cross-cultural understanding and appreciation.Moreover, the rise of modern Chinese culture, such as the popularity of Chinese cuisine, martial arts, and traditional medicine, has also left a mark on English writing. Chinese food, such as dim sum, hot pot, and Peking duck, has become a recurring motif in English literature, reflecting the growing global interest in Chinese culinary delights. Similarly, the depiction of martial arts, such as kung fu and tai chi, in English writing not only showcases the physical prowess of Chinese martial artists but also conveys the spiritual and philosophical aspects of these ancient practices. Furthermore, the portrayal of traditional Chinese medicine, with its emphasis on holistic healing and natural remedies, has added a new dimension to English literature, highlighting the interconnectedness of mind, body, and nature.In conclusion, Chinese elements have enriched English writing in various ways, from traditional symbols and philosophical wisdom to cultural practices and modern phenomena. By incorporating these elements, English writers are able to create works that are not only aesthetically appealing but also culturally resonant, bridging the gap between different literary traditions and fostering a deeper appreciation for the richness of Chinese culture. As the world becomes increasingly interconnected, the fusion of Chinese elements with English writing will continue to inspire and captivate readers around the globe.。
双边跳扩散模型下的奇异期权定价

L ( ) E[ ( a { ma () S t ) e ( xM, a ()) ( ) P T= e m xM xS t} ()] - = ma{ m xI )卜s o s
0《 l r ≤ 一 0《 l r ≤
其 中 ≥S 0 为 0时刻时 给定 的最大 值 。 ()
一
由 K u] 理 中可知 o[ 3 定
LsM,)s I ( ) ] + (, T =e P e T ≥y y d
因此 , 于任 意 的 8 0 对 >
0
』 e
s
:
, T』 I[(>dT T = e MT yd ) s P x) ] + d '  ̄y
一
.
M
=
0
- y -' ,, a -% , y  ̄t y
其 中 ,< 】O< %,< 1 < 0 a<r …< o/ 42 … , ≤p ,i , 1 … , , (i = 。 一步假 设 o> , 2 3 0 j ≤l , n p+ ) 1 进 q q q l 使得 Ee] o。 = [r o 毒 < E[- l ' 设 过程 { ; }{ £ ) 随机 变量 ( 】 1 ̄ g Z R。 £ , ≥O ;≥O及 Z;≥l是独立 的。 易见 , 双指 数跳 扩散模 型是模 型 ( ) 2 中 n l的特 殊情 况 。定义 股票 的投 资 回报 过程 X()lgS t/ ( ) , = t=o ( () O ) 则过 程 x() 描述 为 S t可
,
.
( 5 )
<r n
,
C i证 明了当 6 0时, a > 等式 G() 有 2 + n 2个 不 同的根 FB ,= , … , + , 1< i ̄ i 1 2, n l 且 P 6
Gaugegravity duality

a rXiv:g r-qc/6237v 318Apr261Gauge/gravity duality GARY T.HOROWITZ University of California at Santa Barbara JOSEPH POLCHINSKI KITP,University of California at Santa Barbara Abstract We review the emergence of gravity from gauge theory in the context of AdS/CFT duality.We discuss the evidence for the duality,its lessons for gravitational physics,generalizations,and open questions.1.1Introduction Assertion:Hidden within every non-Abelian gauge theory,even within the weak and strong nuclear interactions,is a theory of quantum gravity.This is one implication of AdS/CFT duality.It was discovered by a circuitous route,involving in particular the relation between black branes and D-branes in string theory.It is an interesting exercise,how-ever,to first try to find a path from gauge theory to gravity as directly as possible.Thus let us imagine that we know a bit about gauge theory and a bit about gravity but nothing about string theory,and ask,how are we to make sense of the assertion?One possibility that comes to mind is that the spin-two graviton mightarise as a composite of two spin-one gauge bosons.This interesting idea would seem to be rigorously excluded by a no-go theorem of Weinberg &Witten (1980).The Weinberg-Witten theorem appears to assume noth-ing more than the existence of a Lorentz-covariant energy momentum tensor,which indeed holds in gauge theory.The theorem does forbid a wide range of possibilities,but (as with several other beautiful and pow-erful no-go theorems)it has at least one hidden assumption that seems so trivial as to escape notice,but which later developments show to be unnecessary.The crucial assumption here is that the graviton moves in the same spacetime as the gauge bosons of which it is made!12Gary T.Horowitz and Joseph PolchinskiThe clue to relax this assumption comes from the holographic prin-ciple(’t Hooft,1993,and Susskind,1995),which suggests that a grav-itational theory should be related to a non-gravitational theory in one fewer dimension.In other words,we mustfind within the gauge theory not just the graviton,but afifth dimension as well:the physics must be local with respect to some additional hidden parameter.Several hints suggest that the role of thisfifth dimension is played by the energy scale of the gauge theory.For example,the renormalization group equation is local with respect to energy:it is a nonlinear evolution equation for the coupling constants as measured at a given energy scale.†In order to make this precise,it is useful to go to certain limits in which thefive-dimensional picture becomes manifest;we will later return to the more general case.Thus we consider four-dimensional gauge theories with the following additional properties:•Large N c.While the holographic principle implies a certain equiva-lence between four-andfive-dimensional theories,it is also true that in many senses a higher dimensional theory has more degrees of free-dom;for example,the one-particle states are labeled by an additional momentum parameter.Thus,in order tofind afifth dimension of macroscopic size,we need to consider gauge theories with many de-grees of freedom.A natural limit of this kind was identified by’t Hooft(1974):if we consider SU(N c)gauge theories,then there is a smooth limit in which N c is taken large with the combination g2YM N c heldfixed.•Strong coupling.Classical Yang-Mills theory is certainly not the same as classical general relativity.If gravity is to emerge from gauge theory, we should expect that it will be in the limit where the gaugefields are strongly quantum mechanical,and the gravitational degrees of freedom arise as effective classicalfields.Thus we must consider the theory with large t Hooft parameter g2YM N c.•Supersymmetry.This is a more technical assumption,but it is a natural corollary to the previous one.Quantumfield theories at strong coupling are prone to severe instabilities;for example,particle-antiparticle pairs can appear spontaneously,and their negative poten-tial energy would exceed their positive rest and kinetic energies.Thus, QED withfine structure constant much greater than1does not exist, even as an effective theory,because it immediately runs into an in-stability in the ultraviolet(known as the Landau pole).The Thirring †This locality was emphasized to us by Shenker,who credits it to Wilson.Gauge/gravity duality3 model provides a simple solvable illustration of the problem:it existsonly below a certain critical coupling(Coleman,1975).Supersym-metric theories however have a natural stability property,because theHamiltonian is the square of a Hermitean supercharge and so boundedbelow.Thus it is not surprising that most examples offield theorieswith interesting strong coupling behavior(i.e.dualities)are super-symmetric.We will therefore start by assuming supersymmetry,butafter understanding this case we can work back to the nonsupersym-metric case.We begin with the most supersymmetric possibility,N=4SU(N c)gauge theory,meaning that there are four copies of the minimal D=4supersymmetry algebra.The assumption of N=4supersymmetry hasa useful bonus in that the beta function vanishes,the coupling does notrun.Most gauge theories have running couplings,so that the strongcoupling required by the previous argument persists only in a very nar-row range of energies,becoming weak on one side and blowing up onthe other.In the N=4gauge theory the coupling remains strong andconstant over an arbitrarily large range,and so we can have a largefifthdimension.The vanishing beta function implies that the classical conformal in-variance of the Yang-Mills theory survives quantization:it is a conformalfield theory(CFT).In particular,the theory is invariant under rigid scaletransformations xµ→λxµforµ=0,1,2,3.Since we are associating thefifth coordinate r with energy scale,it must tranform inversely tothe length scale,r→r/λ.The most general metric invariant under thisscale invariance and the ordinary Poincar´e symmetries isds2=r2r2dr2(1.1)for some constantsℓandℓ′;by a multiplicative redefinition of r we can setℓ′=ℓ.Thus our attempt to make sense of the assertion at the beginning has led us(with liberal use of hindsight)to the following conjecture:D=4,N=4,SU(N c)gauge theory is equivalent to a grav-itational theory infive-dimensional anti-de Sitter(AdS)space.Indeed, this appears to be true.In the next section we will make this statement more precise,and discuss the evidence for it.In thefinal section we will discuss various lessons for quantum gravity,generalizations,and open questions.4Gary T.Horowitz and Joseph Polchinski1.2AdS/CFT dualityLet us define more fully the two sides of the duality.†The gauge theory can be written in a compact way by starting with the D=10Lagrangian density for an SU(N c)gaugefield and a16component Majorana-Weyl spinor,both in the adjoint(N c×N c matrix)representation:L=1Gauge/gravity duality5 completely crazy comes from comparing the symmetries.The D=4, N=4,SU(N c)super-Yang-Mills theory has an SO(4,2)symmetrycoming from conformal invariance and an SO(6)symmetry coming from rotation of the scalars.This agrees with the geometric symmetries of AdS5×S5.On both sides there are also32supersymmetries.Again on the gravitational side these are geometric,arising as Killing spinors on the AdS5×S5spacetime.On the gauge theory side they include the 16‘ordinary’supersymmetries of the N=4algebra,and16additional supersymmetries as required by the conformal algebra.The precise(though still not fully complete)statement is that the IIB supergravity theory in a space whose geometry is asymptotically AdS5×S5is dual to the D=4,N=4,SU(N c)gauge theory.The metric(1.1) describes only a Poincar´e patch of AdS spacetime,and the gauge theory lives on R4.It is generally more natural to consider the fully extended global AdS space,in which case the dual gauge theory lives on S3×R. In each case the gauge theory lives on the conformal boundary of the gravitational spacetime(r→∞in the Poincar´e coordinates),which will give us a natural dictionary for the observables.The initial checks of this duality concerned perturbations of AdS5×S5. It was shown that all linearized supergravity states have corresponding states in the gauge theory(Witten,1998a).In particular,the global time translation in the bulk is identified with time translation in the field theory,and the energies of states in thefield theory and string theory agree.For perturbations of AdS5×S5,one can reconstruct the background spacetime from the gauge theory as follows.Fields on S5 can be decomposed into spherical harmonics,which can be described as symmetric traceless tensors on R6:T i···j X i···X j.Restricted to the unit sphere one gets a basis of functions.Recall that the gauge theory has six scalars and the SO(6)symmetry of rotating theϕi.So the operators T i···jϕi···ϕj give information about position on S5.Four of the remaining directions are explicitly present in the gauge theory,and the radial direction corresponds to the energy scale in the gauge theory. In the gauge theory the expectation values of local operators(gauge invariant products of the N=4fields and their covariant derivatives) provide one natural set of observables.It is convenient to work with the generating functional for these expectation values by shifting the Lagrangian densityL(x)→L(x)+ I J I(x)O I(x),(1.3) where O I is some basis of local operators and J I(x)are arbitrary func-6Gary T.Horowitz and Joseph Polchinskitions.Since we are taking products of operators at a point,we are perturbing the theory in the ultraviolet,which according to the energy-radius relation maps to the AdS boundary.Thus the duality dictionary relates the gauge theory generating functional to a gravitational theory in which the boundary conditions at infinity are perturbed in a specified way(Gubser et al.,1998,and Witten,1998a).As a further check on the duality,all three-point interactions were shown to agree(Lee et al., 1998).The linearized supergravity excitations map to gauge invariant states of the gauge bosons,scalars,and fermions,but in fact only to a small subset of these;in particular,all the supergravity states live in special small multiplets of the superconformal symmetry algebra.Thus the dual to the gauge theory contains much more than supergravity.The identity of the additional degrees of freedom becomes particularly clear if one looks at highly boosted states,those having large angular momentum on S5and/or AdS5(Berenstein et al.,2002,and Gubser et al.,2002).The fields of the gauge theory then organize naturally into one-dimensional structures,coming from the Yang-Mills large-N c trace:they correspond to the excited states of strings.In some cases,one can even construct a two dimensional sigma model directly from the gauge theory and show that it agrees(at large boost)with the sigma model describing strings moving in AdS5×S5(Kruczenski,2004).Thus,by trying to make sense of the assertion at the beginning,we are forced to‘discover’string theory.We can now state the duality in its full form(Maldacena,1998a):Four-dimensional N=4supersymmetric SU(N c)gauge theory is equivalent to IIB string theory with AdS5×S5boundary conditions. The need for strings(though not the presence of gravity!)was already anticipated by’t Hooft(1974),based on the planar structure of the large-N c Yang-Mills perturbation theory;the AdS/CFT duality puts this into a precise form.It alsofits with the existence of another important set of gauge theory observables,the one-dimensional Wilson loops.The Wilson loop can be thought of as creating a string at the AdS5boundary, whose world-sheet then extends into the interior(Maldacena,1998b,and Rey&Yee,2001).See also Polyakov(1987,1999)for other perspectives on gauge/string duality and the role of thefifth dimension.We now drop the pretense of not knowing string theory,and outline the original argument for the duality in Maldacena(1998a).He con-sidered a stack of N c parallel D3-branes on top of each other.EachGauge/gravity duality7 D3-brane couples to gravity with a strength proportional to the dimen-sionless string coupling g s,so the distortion of the metric by the branesis proportional to g s N c.When g s N c≪1the spacetime is nearlyflat and there are two types of string excitations.There are open strings on thebrane whose low energy modes are described by a U(N c)gauge theory.There are also closed strings away from the brane.When g s N c≫1,the backreaction is important and the metric describes an extremal black 3-brane.This is a generalization of a black hole appropriate for a three dimensional extended object.It is extremal with respect to the charge carried by the3-branes,which sources thefive form F5.Near the hori-zon,the spacetime becomes a product of S5and AdS5.(This is directly analogous to the fact that near the horizon of an extremal Reissner-Nordstrom black hole,the spacetime is AdS2×S2.)String states near the horizon are strongly redshifted and have very low energy as seen asymptotically.In a certain low energy limit,one can decouple these strings from the strings in the asymptoticallyflat region.At weak cou-pling,g s N c≪1,this same limit decouples the excitations of the3-branes from the closed strings.Thus the low energy decoupled physics is de-scribed by the gauge theory at small g s and by the AdS5×S5closed string theory at large g s,and the simplest conjecture is that these are the same theory as seen at different values of the coupling.†This con-jecture resolved a puzzle,the fact that very different gauge theory and gravity calculations were found to give the same answers for a variety of string-brane interactions.In the context of string theory we can relate the parameters on thetwo sides of the duality.In the gauge theory we have g2YM and N c.Theknown D3-brane Lagrangian determines the relation of couplings,g2YM=4πg s.Further,each D3-brane is a source for thefive-formfield strength,so on the string side N c is determined by S5F5;this integratedflux is quantized by a generalization of Dirac’s argument for quantization of the flux S2F2of a magnetic monopole.The supergravityfield equations give a relation between thisflux and the radii of curvature of the AdS5 and S5spaces,both being given byℓ=(4πg s N c)1/4ℓs.(1.4) Hereℓs is the fundamental length scale of string theory,related to the string tensionµbyµ−1=2πℓ2s.Notice that the spacetime radii are large in string units(and so the curvature is small)precisely when the’t Hooft †The U(1)factor in U(N c)=SU(N c)×U(1)also decouples:it is Abelian and does not feel the strong gauge interactions.8Gary T.Horowitz and Joseph Polchinskicoupling4πg s N c=g2YM N c is large,in keeping with the heuristic argu-ments that we made in the introduction.It is also instructive to express the AdS radius entirely in gravitational variables.The ten-dimensional gravitational coupling is G∼g2sℓ8s,up to a numerical constant.Thusℓ∼N1/4c G1/8,G∼ℓ8ℓ2+1−r20ℓ2+1−r20Gauge/gravity duality9 isS BH=Ag2sℓ8s∼T3Hℓ114S YM(Gubser et al.,1996).The numerical disagreement is not surprising,as the Yang-Mills calculation is for an ideal gas,and at large g s the Yang-Mills degrees of freedom are interacting.Thus one expects a relation of the form S BH=f(g s N c)S YM,ideal,where f(0)=1;the above calculation implies that f(∞)=34,but thefirst correction has been calculated both at weakand strong coupling and is consistent with f(g s N c)interpolating in a rather smooth way.Hawking&Page(1983)showed that for thermal AdS boundary con-ditions there is a phase transition:below a transition temperature of order1/ℓthe dominant configuration is not the black hole but a gas of particles in AdS space.The low temperature geometry has no horizon and so its entropy comes only from the ordinary statistical mechanics of the gas.The same transition occurs in the gauge theory(Witten, 1998b).The N=4gauge theory on S3has an analog of a confinement transition.At low temperature one has a thermal ensemble of gauge-invariant degrees of freedom,whose entropy therefore scales as N0c,and at high temperature one has the N2c behavior found above—the same scalings as on the gravitational side.There is another test one can perform with the gauge theory atfi-nite temperature.At long wavelengths,one can use a hydrodynamic approximation and think of this as afluid(for a recent overview see Kovtun et al.,2003).It is then natural to ask:What is the speed of sound waves?Conformal invariance implies that the stress energy ten-sor is traceless,so p=ρ/3which implies that v=1/√10Gary T.Horowitz and Joseph Polchinskiseem to be difficult since the bulk does not seem to have any preferred speed other than the speed of light.But recent work has shown that the answer is yes.The AdS/CFT duality also gives an interesting perspective on the black hole membrane paradigm(Thorne et al.,1986).The black hole horizon is known to have many of the properties of a dissipative sys-tem.On the dual side it is a dissipative system,the hot gauge theory. One can thus compute such hydrodynamic quantities such as the shear viscosity.These are hard to check since they are difficult to calculate directly in the strongly coupled thermal gauge theory,but,rather re-markably,the numerical agreement with the observed properties of the real quark-gluon plasma at RHIC is better than for conventionalfield theory calculations(for a discussion see Blau,2005).There is also afield theory interpretation of black hole quasinormal modes(Horowitz&Hubeny2000).A perturbation of the black hole decays with a characteristic time set by the imaginary part of the low-est quasinormal mode.This should correspond to the timescale for the gauge theory to return to thermal equilibrium.One can show that the quasinormal mode frequencies are poles in the retarded Green’s func-tion of a certain operator in the gauge theory.The particular operator depends on the type offield used to perturb the black hole(Kovtun& Starinets,2005).Finally,consider the formation and evaporation of a small black hole in a spacetime which is asymptotically AdS5×S5.By the AdS/CFT correspondence,this process is described by ordinary unitary evolution in the gauge theory.So black hole evaporation does not violate quan-tum mechanics:information is preserved.This also provides an indirect argument against the existence of a‘bounce’at the black hole singular-ity,because the resulting disconnected universe would presumably carry away information.1.3.2Background independence and emergenceThe AdS/CFT system is entirely embedded in the framework of quan-tum mechanics.On the gauge theory side we have an explicit Hamil-tonian,and states which we can think of as gauge invariant functionals of thefields.Thus the gravitational theory on the other side is quan-tum mechanical as well.In particular the metricfluctuates freely except at the AdS boundary.One is not restricted to perturbations about a particular background.Gauge/gravity duality11 This is clearly illustrated by a rich set of examples which provide a detailed map between a class of nontrivial asymptotically AdS5×S5supergravity solutions and a class of states in the gauge theory(Lin et al.,2004).These states and geometries both preserve half of the supersymmetry of AdS5×S5itself.On thefield theory side,one restricts tofields that are independent of S3and hence reduce to N c×N c matrices. In fact,all the states are created by a single complex matrix,so can be described by a one-matrix model.This theory can be quantized exactly in terms of free fermions,and the states can be labeled by a arbitrary closed curve(the Fermi surface)on a plane.On the gravity side,one considers solutions to ten dimensional supergravity involving just the metric and self-dualfive form F5.Thefield equations are simply dF5=0 andR MN=F MP QRS F N P QRS(1.9) There exists a large class of stationary solutions to(1.9),which have an SO(4)×SO(4)symmetry and can be obtained by solving a linear equation.These solutions are nonsingular,have no event horizons,but can have complicated topology.They are also labeled by arbitrary closed curves on a plane.This provides a precise way to map states in thefield theory into bulk geometries.Only for some“semi-classical”states is the curvature below the Planck scale everywhere,but the matrix/free fermion description readily describes all the states,of all topologies, within a single Hilbert space.Thus the gauge theory gives a representation of quantum gravity that is background independent almost everywhere—-that is,everywhere ex-cept the boundary.Conventional string perturbation theory constructs string amplitudes as an asymptotic expansion around a given spacetime geometry;here we have an exact quantum mechanical construction for which the conventional expansion generates the asymptotics.All lo-cal phenomena of quantum gravity,such as formation and evaporation of black holes,the interaction of quanta with Planckian energies,and even transitions that change topology,are described by the gauge the-ory.However,the boundary conditions do have the important limitation that most cosmological situations,and most compactifications of string theory,cannot be described;we will return to these points later.To summarize,AdS/CFT duality is an example of emergent gravity, emergent spacetime,and emergent general coordinate invariance.But it is also an example of emergent strings!We should note that the terms‘gauge/gravity duality’and‘gauge/string duality’are often used,12Gary T.Horowitz and Joseph Polchinskiboth to reflect these emergent properties and also the fact that(as weare about the see)the duality generalizes to gravitational theories withcertain other boundary conditions,and tofield theories that are notconformally invariant.Let us expand somewhat on the emergence of general coordinate in-variance.The AdS/CFT duality is a close analog to the phenomenonof emergent gauge symmetry(e.g.D’Adda et al.,1978,and Baskaran&Anderson,1988).For example,in some condensed matter systems inwhich the starting point has only electrons with short-ranged interac-tions,there are phases where the electron separates into a new fermionand boson,e(x)=b(x)f†(x).(1.10) However,the newfields are redundant:there is a gauge transformationb(x)→e iλ(x)b(x),f(x)→e iλ(x)f(x),which leaves the physical elec-tronfield invariant.This new gauge invariance is clearly emergent:it iscompletely invisible in terms of the electronfield appearing in the orig-inal description of the theory.†Similarly,the gauge theory variables ofAdS/CFT are trivially invariant under the bulk diffeomorphisms,whichare entirely invisible in the gauge theory(the gauge theoryfields dotransform under the asymptotic symmetries of AdS5×S5,but these are ADM symmetries,not gauge redundancies).Of course we can alwaysin general relativity introduce a set of gauge-invariant observables bysetting up effectively a system of rods and clocks,so to this extent thenotion of emergence is imprecise,but it carries the connotation that thedynamics can be expressed in a simple way in terms of the invariantvariables,as the case in AdS/CFT.‡1.3.3GeneralizationsThus far we have considered only the most well-studied example ofgauge/gravity duality:D=4,N=4,Yang-Mills⇔string theorywith AdS5×S5boundary conditions.Let us now ask how much more general this phenomenon is(again,for details see the review by Aharony et al.,2000).†This‘statistical’gauge invariance is not to be confused with the ordinary electro-magnetic gauge invariance,which does act on the electron.‡Note that on the gauge theory side there is still the ordinary Yang-Mills gauge redundancy,which is more tractable than general coordinate invariance(it does not act on spacetime).In fact in most examples of duality there are gauge symmetries on both sides and these are unrelated to each other:the duality pertains only to the physical quantities.Gauge/gravity duality13 First,we imagine perturbing the theory we have already studied, adding additional terms(such as masses for some of thefields)to the gauge theory action.This is just a special case of the modification(1.3), such that the functions J I(x)=g I are independent of position.Thus we already have the dictionary,that the the dual theory is given by IIB string theory in a spacetime with some perturbation of the AdS5×S5 boundary conditions.In general,the perturbation of the gauge theory will break conformal invariance,so that the physics depends on energy scale.In quantum field theory there is a standard procedure for integrating out high en-ergy degrees of freedom and obtaining an effective theory at low energy. This is known as renormalization group(RG)flow.If one starts with a conformalfield theory at high energy,the RGflow is trivial.The low energy theory looks the same as the high energy theory.This is because there is no intrinsic scale.But if we perturb the theory,the RGflow is nontrivial and we obtain a different theory at low energies.There are two broad possibilities:either some degrees of freedom remain massless and we approach a new conformal theory at low energy,or allfields become massive and the low energy limit is trivial.Since the energy scale corresponds to the radius,this RGflow in the boundaryfield theory should correspond to radial dependence in the bulk.Let us expand a bit on the relation between radial coordinate and energy(we will make this argument in Poincar´e coordinates,since the perturbed gauge theories are usually studied on R4).The AdS geom-etry(1.1)is warped:in Poincare coordinates,the fourflat dimensions experience a gravitational redshift that depends onfifth coordinate,just as in Randall-Sundrum compactification.Consequently the conserved Killing momentum pµ(Noether momentum in the gauge theory)is re-lated to the local inertial momentum˜pµbyrpµ=14Gary T.Horowitz and Joseph Polchinskioffin such a way that the warp factor(which is r/ℓin AdS spacetime) has a lower bound.The former clearly corresponds to a new conformal theory,while the latter would imply a mass gap,by the argument fol-lowing eq.(1.11).In the various examples,onefinds that the nature of the solution correctly reflects the low energy physics as expected from gauge theory arguments;there is also more detailed numerical agree-ment(Freedman et al.,1999).So the classical Einstein equation knows a lot about RGflows in quantumfield theory.A notable example is the case where one gives mass to all the scalars and fermions,leaving only the gaugefields massless in the Lagrangian. One then expects the gauge theory toflow to strong coupling and pro-duce a mass gap,and this is what is found in the supergravity solution. Further,the gauge theory should confine,and indeed in the deformed geometry a confining area law is found for the Wilson loop(but still a perimeter law for the’t Hooft loop,again as expected).In other ex-amples one alsofinds chiral symmetry breaking,as expected in strongly coupled gauge theories(Klebanov&Strassler,2000).As a second generalization,rather than a deformation of the geometry we can make a big change,replacing S5with any other Einstein space; the simplest examples would be S5identified by some discrete subgroup of its SO(6)symmetry.The product of the Einstein space with AdS5 still solves thefield equations(at least classically),so there should be a conformally invariant dual.These duals are known in a very large class of examples;characteristically they are quiver gauge theories,a product of SU(N1)×...×SU(N k)with matterfields transforming as adjoints and bifundamentals(one can also get orthogonal and symplectic factors). As a third generalization,we can start with D p-branes for other val-ues of p,or combinations of branes of different dimensions.These lead to other examples of gauge-gravity duality forfield theories in various dimensions,many of which are nonconformal.The case p=0is the BFSS matrix model,although the focus in that case is on a different set of observables,the scattering amplitudes for the D0-branes themselves.A particularly interesting system is D1-branes plus D5-branes,leading to the near-horizon geometry AdS3×S3×T4.This case has at least one advantage over AdS5×S5.The entropy of large black holes can now be reproduced exactly,including the numerical coefficient.This is related to the fact that a black hole in AdS3is a BTZ black hole which is locally AdS3everywhere.Thus when one extrapolates to small coupling,one does not modify the geometry with higher curvature corrections.We have discussed modifications of the gauge theory’s Hamiltonian,。
nametrendswhat

FA S H I O N FAVO R I T E S
Girls: Hannah, Abigail, Madeline, Sophia Boys: Elijah, Caleb, Oliver, Sebastian
FRESH OPTIONS
Girls: Susannah, Rafaela, Beatrice, Lavinia Boys: Japheth, Matthias, Phineas, Benedict See more under: Antique Charm, Biblical, Exotic Traditionals, Ladies and GentlemenS Nhomakorabearnames
Surnames are now a top source for first names, in a style that recalls the 1900s heyday of names like Ellsworth, Palmer, and Jennings. Unlike that earlier generation, though, today’s surnames are used for boys and girls alike. One of the hottest styles for girls is long, masculine-sounding surnames that shorten to familiar girlish nicknames. Emerson (Emmy), Cassidy (Cassie), and Addison (Addie) are all rising. On the flip side, if you’re looking for a fresh surname idea for boys that will stay firmly masculine, try rugged tradesman names, or surnames that contract to male nicknames.
Adaptive tracking control of uncertain MIMO nonlinear systems with input constraints

article
info
abstract
In this paper, adaptive tracking control is proposed for a class of uncertain multi-input and multi-output nonlinear systems with non-symmetric input constraints. The auxiliary design system is introduced to analyze the effect of input constraints, and its states are used to adaptive tracking control design. The spectral radius of the control coefficient matrix is used to relax the nonsingular assumption of the control coefficient matrix. Subsequently, the constrained adaptive control is presented, where command filters are adopted to implement the emulate of actuator physical constraints on the control law and virtual control laws and avoid the tedious analytic computations of time derivatives of virtual control laws in the backstepping procedure. Under the proposed control techniques, the closed-loop semi-global uniformly ultimate bounded stability is achieved via Lyapunov synthesis. Finally, simulation studies are presented to illustrate the effectiveness of the proposed adaptive tracking control. © 2011 Elsevier Ltd. All rights reserved.
英语作文超市购物的利与弊

英语作文超市购物的利与弊In the labyrinthine aisles of the supermarket, I embark on a weekly ritual that both enchants and perplexes me. The symphony of bustling shoppers, the kaleidoscope of vibrant produce, and the tantalizing aromas create an immersive experience that beckons me to explore its depths. Yet, as I navigate this modern-day bazaar, I find myself grappling with the paradoxical nature of supermarket shopping – its undeniable conveniences juxtaposed with its potential pitfalls.On the one hand, supermarkets offer an unparalleled level of convenience. They are havens of accessibility, open at all hours, and stocked with an astonishing array of products from every corner of the globe. Gone are the days of arduous market visits, haggling over prices, and lugging heavy baskets home. With a few swipes of a card, I can procure everything from fresh fruits and vegetables to frozen dinners, cleaning supplies, and even electronics. The sheer abundance and ease of access are undeniable boons to our busy lifestyles.Moreover, supermarkets have become veritable culinaryplaygrounds. They offer a tantalizing selection of exotic ingredients, gourmet delicacies, and organic produce that would have been unimaginable just a few decades ago. This culinary diversity has ignited a passion for cooking and experimentation, allowing us to explore new flavors and cuisines from the comfort of our own homes. The supermarket has transformed into a culinary adventure, where we can embark on culinary journeys without ever leaving our neighborhood.However, the allure of convenience and culinary delights comes at a price. Supermarkets have also become breeding grounds for processed foods, sugary drinks, and unhealthy snacks. The aisles are lined with temptations that cater to our impulsive cravings and undermine our nutritional well-being. The abundance of choice can be overwhelming, making it challenging to resist the siren call of unhealthy options.Furthermore, the environmental impact of supermarket shopping cannot be ignored. The vast majority of products are packaged in plastic or other non-biodegradable materials, contributing to the staggering amount of wastegenerated by our consumerist society. The transportation of goods from far-flung corners of the world also takes a toll on the environment, emitting greenhouse gases and exacerbating climate change.As I stand at the checkout counter, contemplating my purchases, I am struck by the duality of supermarket shopping. It is a place of convenience, culinary exploration, and environmental concern. It is a reflection of our modern world, where progress and challenges coexist. Ultimately, the decision of whether to embrace or eschew supermarket shopping is a personal one. There is no doubt that it has revolutionized the way we acquire food and other necessities. However, it is essential to be mindful of the potential pitfalls and to make informed choices that align with our values and priorities.For me, supermarket shopping will continue to be a bittersweet experience. I will revel in the convenience and culinary delights it offers while striving to minimize my environmental footprint and make healthier choices. By striking a balance between indulgence and responsibility, I hope to navigate the aisles of the supermarket with a clearconscience and a sense of purpose.。
An Overview of Recent Progress in the Study of Distributed Multi-agent Coordination

An Overview of Recent Progress in the Study of Distributed Multi-agent CoordinationYongcan Cao,Member,IEEE,Wenwu Yu,Member,IEEE,Wei Ren,Member,IEEE,and Guanrong Chen,Fellow,IEEEAbstract—This article reviews some main results and progress in distributed multi-agent coordination,focusing on papers pub-lished in major control systems and robotics journals since 2006.Distributed coordination of multiple vehicles,including unmanned aerial vehicles,unmanned ground vehicles and un-manned underwater vehicles,has been a very active research subject studied extensively by the systems and control community. The recent results in this area are categorized into several directions,such as consensus,formation control,optimization, and estimation.After the review,a short discussion section is included to summarize the existing research and to propose several promising research directions along with some open problems that are deemed important for further investigations.Index Terms—Distributed coordination,formation control,sen-sor networks,multi-agent systemI.I NTRODUCTIONC ONTROL theory and practice may date back to thebeginning of the last century when Wright Brothers attempted theirfirst testflight in1903.Since then,control theory has gradually gained popularity,receiving more and wider attention especially during the World War II when it was developed and applied tofire-control systems,missile nav-igation and guidance,as well as various electronic automation devices.In the past several decades,modern control theory was further advanced due to the booming of aerospace technology based on large-scale engineering systems.During the rapid and sustained development of the modern control theory,technology for controlling a single vehicle, albeit higher-dimensional and complex,has become relatively mature and has produced many effective tools such as PID control,adaptive control,nonlinear control,intelligent control, This work was supported by the National Science Foundation under CAREER Award ECCS-1213291,the National Natural Science Foundation of China under Grant No.61104145and61120106010,the Natural Science Foundation of Jiangsu Province of China under Grant No.BK2011581,the Research Fund for the Doctoral Program of Higher Education of China under Grant No.20110092120024,the Fundamental Research Funds for the Central Universities of China,and the Hong Kong RGC under GRF Grant CityU1114/11E.The work of Yongcan Cao was supported by a National Research Council Research Associateship Award at AFRL.Y.Cao is with the Control Science Center of Excellence,Air Force Research Laboratory,Wright-Patterson AFB,OH45433,USA.W.Yu is with the Department of Mathematics,Southeast University,Nanjing210096,China and also with the School of Electrical and Computer Engineering,RMIT University,Melbourne VIC3001,Australia.W.Ren is with the Department of Electrical Engineering,University of California,Riverside,CA92521,USA.G.Chen is with the Department of Electronic Engineering,City University of Hong Kong,Hong Kong SAR,China.Copyright(c)2009IEEE.Personal use of this material is permitted. However,permission to use this material for any other purposes must be obtained from the IEEE by sending a request to pubs-permissions@.and robust control methodologies.In the past two decades in particular,control of multiple vehicles has received increas-ing demands spurred by the fact that many benefits can be obtained when a single complicated vehicle is equivalently replaced by multiple yet simpler vehicles.In this endeavor, two approaches are commonly adopted for controlling multiple vehicles:a centralized approach and a distributed approach. The centralized approach is based on the assumption that a central station is available and powerful enough to control a whole group of vehicles.Essentially,the centralized ap-proach is a direct extension of the traditional single-vehicle-based control philosophy and strategy.On the contrary,the distributed approach does not require a central station for control,at the cost of becoming far more complex in structure and organization.Although both approaches are considered practical depending on the situations and conditions of the real applications,the distributed approach is believed more promising due to many inevitable physical constraints such as limited resources and energy,short wireless communication ranges,narrow bandwidths,and large sizes of vehicles to manage and control.Therefore,the focus of this overview is placed on the distributed approach.In distributed control of a group of autonomous vehicles,the main objective typically is to have the whole group of vehicles working in a cooperative fashion throughout a distributed pro-tocol.Here,cooperative refers to a close relationship among all vehicles in the group where information sharing plays a central role.The distributed approach has many advantages in achieving cooperative group performances,especially with low operational costs,less system requirements,high robustness, strong adaptivity,andflexible scalability,therefore has been widely recognized and appreciated.The study of distributed control of multiple vehicles was perhapsfirst motivated by the work in distributed comput-ing[1],management science[2],and statistical physics[3]. In the control systems society,some pioneering works are generally referred to[4],[5],where an asynchronous agree-ment problem was studied for distributed decision-making problems.Thereafter,some consensus algorithms were studied under various information-flow constraints[6]–[10].There are several journal special issues on the related topics published af-ter2006,including the IEEE Transactions on Control Systems Technology(vol.15,no.4,2007),Proceedings of the IEEE (vol.94,no.4,2007),ASME Journal of Dynamic Systems, Measurement,and Control(vol.129,no.5,2007),SIAM Journal of Control and Optimization(vol.48,no.1,2009),and International Journal of Robust and Nonlinear Control(vol.21,no.12,2011).In addition,there are some recent reviewsand progress reports given in the surveys[11]–[15]and thebooks[16]–[23],among others.This article reviews some main results and recent progressin distributed multi-agent coordination,published in majorcontrol systems and robotics journals since2006.Due to space limitations,we refer the readers to[24]for a more completeversion of the same overview.For results before2006,thereaders are referred to[11]–[14].Specifically,this article reviews the recent research resultsin the following directions,which are not independent but actually may have overlapping to some extent:1.Consensus and the like(synchronization,rendezvous).Consensus refers to the group behavior that all theagents asymptotically reach a certain common agreementthrough a local distributed protocol,with or without predefined common speed and orientation.2.Distributed formation and the like(flocking).Distributedformation refers to the group behavior that all the agents form a pre-designed geometrical configuration throughlocal interactions with or without a common reference.3.Distributed optimization.This refers to algorithmic devel-opments for the analysis and optimization of large-scaledistributed systems.4.Distributed estimation and control.This refers to dis-tributed control design based on local estimation aboutthe needed global information.The rest of this article is organized as follows.In Section II,basic notations of graph theory and stochastic matrices are introduced.Sections III,IV,V,and VI describe the recentresearch results and progress in consensus,formation control, optimization,and estimation.Finally,the article is concludedby a short section of discussions with future perspectives.II.P RELIMINARIESA.Graph TheoryFor a system of n connected agents,its network topology can be modeled as a directed graph denoted by G=(V,W),where V={v1,v2,···,v n}and W⊆V×V are,respectively, the set of agents and the set of edges which directionallyconnect the agents together.Specifically,the directed edgedenoted by an ordered pair(v i,v j)means that agent j can access the state information of agent i.Accordingly,agent i is a neighbor of agent j.A directed path is a sequence of directed edges in the form of(v1,v2),(v2,v3),···,with all v i∈V.A directed graph has a directed spanning tree if there exists at least one agent that has a directed path to every other agent.The union of a set of directed graphs with the same setof agents,{G i1,···,G im},is a directed graph with the sameset of agents and its set of edges is given by the union of the edge sets of all the directed graphs G ij,j=1,···,m.A complete directed graph is a directed graph in which each pair of distinct agents is bidirectionally connected by an edge,thus there is a directed path from any agent to any other agent in the network.Two matrices are used to represent the network topology: the adjacency matrix A=[a ij]∈R n×n with a ij>0if (v j,v i)∈W and a ij=0otherwise,and the Laplacian matrix L=[ℓij]∈R n×n withℓii= n j=1a ij andℓij=−a ij,i=j, which is generally asymmetric for directed graphs.B.Stochastic MatricesA nonnegative square matrix is called(row)stochastic matrix if its every row is summed up to one.The product of two stochastic matrices is still a stochastic matrix.A row stochastic matrix P∈R n×n is called indecomposable and aperiodic if lim k→∞P k=1y T for some y∈R n[25],where 1is a vector with all elements being1.III.C ONSENSUSConsider a group of n agents,each with single-integrator kinematics described by˙x i(t)=u i(t),i=1,···,n,(1) where x i(t)and u i(t)are,respectively,the state and the control input of the i th agent.A typical consensus control algorithm is designed asu i(t)=nj=1a ij(t)[x j(t)−x i(t)],(2)where a ij(t)is the(i,j)th entry of the corresponding ad-jacency matrix at time t.The main idea behind(2)is that each agent moves towards the weighted average of the states of its neighbors.Given the switching network pattern due to the continuous motions of the dynamic agents,coupling coefficients a ij(t)in(2),hence the graph topologies,are generally time-varying.It is shown in[9],[10]that consensus is achieved if the underlying directed graph has a directed spanning tree in some jointly fashion in terms of a union of its time-varying graph topologies.The idea behind consensus serves as a fundamental principle for the design of distributed multi-agent coordination algo-rithms.Therefore,investigating consensus has been a main research direction in the study of distributed multi-agent co-ordination.To bridge the gap between the study of consensus algorithms and many physical properties inherited in practical systems,it is necessary and meaningful to study consensus by considering many practical factors,such as actuation,control, communication,computation,and vehicle dynamics,which characterize some important features of practical systems.This is the main motivation to study consensus.In the following part of the section,an overview of the research progress in the study of consensus is given,regarding stochastic network topologies and dynamics,complex dynamical systems,delay effects,and quantization,mainly after2006.Several milestone results prior to2006can be found in[2],[4]–[6],[8]–[10], [26].A.Stochastic Network Topologies and DynamicsIn multi-agent systems,the network topology among all vehicles plays a crucial role in determining consensus.The objective here is to explicitly identify necessary and/or suffi-cient conditions on the network topology such that consensus can be achieved under properly designed algorithms.It is often reasonable to consider the case when the network topology is deterministic under ideal communication chan-nels.Accordingly,main research on the consensus problem was conducted under a deterministicfixed/switching network topology.That is,the adjacency matrix A(t)is deterministic. Some other times,when considering random communication failures,random packet drops,and communication channel instabilities inherited in physical communication channels,it is necessary and important to study consensus problem in the stochastic setting where a network topology evolves according to some random distributions.That is,the adjacency matrix A(t)is stochastically evolving.In the deterministic setting,consensus is said to be achieved if all agents eventually reach agreement on a common state. In the stochastic setting,consensus is said to be achieved almost surely(respectively,in mean-square or in probability)if all agents reach agreement on a common state almost surely (respectively,in mean-square or with probability one).Note that the problem studied in the stochastic setting is slightly different from that studied in the deterministic setting due to the different assumptions in terms of the network topology. Consensus over a stochastic network topology was perhaps first studied in[27],where some sufficient conditions on the network topology were given to guarantee consensus with probability one for systems with single-integrator kinemat-ics(1),where the rate of convergence was also studied.Further results for consensus under a stochastic network topology were reported in[28]–[30],where research effort was conducted for systems with single-integrator kinematics[28],[29]or double-integrator dynamics[30].Consensus for single-integrator kine-matics under stochastic network topology has been exten-sively studied in particular,where some general conditions for almost-surely consensus was derived[29].Loosely speaking, almost-surely consensus for single-integrator kinematics can be achieved,i.e.,x i(t)−x j(t)→0almost surely,if and only if the expectation of the network topology,namely,the network topology associated with expectation E[A(t)],has a directed spanning tree.It is worth noting that the conditions are analogous to that in[9],[10],but in the stochastic setting. In view of the special structure of the closed-loop systems concerning consensus for single-integrator kinematics,basic properties of the stochastic matrices play a crucial role in the convergence analysis of the associated control algorithms. Consensus for double-integrator dynamics was studied in[30], where the switching network topology is assumed to be driven by a Bernoulli process,and it was shown that consensus can be achieved if the union of all the graphs has a directed spanning tree.Apparently,the requirement on the network topology for double-integrator dynamics is a special case of that for single-integrator kinematics due to the difference nature of thefinal states(constantfinal states for single-integrator kinematics and possible dynamicfinal states for double-integrator dynamics) caused by the substantial dynamical difference.It is still an open question as if some general conditions(corresponding to some specific algorithms)can be found for consensus with double-integrator dynamics.In addition to analyzing the conditions on the network topology such that consensus can be achieved,a special type of consensus algorithm,the so-called gossip algorithm[31],[32], has been used to achieve consensus in the stochastic setting. The gossip algorithm can always guarantee consensus almost surely if the available pairwise communication channels satisfy certain conditions(such as a connected graph).The way of network topology switching does not play any role in the consideration of consensus.The current study on consensus over stochastic network topologies has shown some interesting results regarding:(1) consensus algorithm design for various multi-agent systems,(2)conditions of the network topologies on consensus,and(3)effects of the stochastic network topologies on the con-vergence rate.Future research on this topic includes,but not limited to,the following two directions:(1)when the network topology itself is stochastic,how to determine the probability of reaching consensus almost surely?(2)compared with the deterministic network topology,what are the advantages and disadvantages of the stochastic network topology,regarding such as robustness and convergence rate?As is well known,disturbances and uncertainties often exist in networked systems,for example,channel noise,commu-nication noise,uncertainties in network parameters,etc.In addition to the stochastic network topologies discussed above, the effect of stochastic disturbances[33],[34]and uncertain-ties[35]on the consensus problem also needs investigation. Study has been mainly devoted to analyzing the performance of consensus algorithms subject to disturbances and to present-ing conditions on the uncertainties such that consensus can be achieved.In addition,another interesting direction in dealing with disturbances and uncertainties is to design distributed localfiltering algorithms so as to save energy and improve computational efficiency.Distributed localfiltering algorithms play an important role and are more effective than traditional centralizedfiltering algorithms for multi-agent systems.For example,in[36]–[38]some distributed Kalmanfilters are designed to implement data fusion.In[39],by analyzing consensus and pinning control in synchronization of complex networks,distributed consensusfiltering in sensor networks is addressed.Recently,Kalmanfiltering over a packet-dropping network is designed through a probabilistic approach[40]. Today,it remains a challenging problem to incorporate both dynamics of consensus and probabilistic(Kalman)filtering into a unified framework.plex Dynamical SystemsSince consensus is concerned with the behavior of a group of vehicles,it is natural to consider the system dynamics for practical vehicles in the study of the consensus problem. Although the study of consensus under various system dynam-ics is due to the existence of complex dynamics in practical systems,it is also interesting to observe that system dynamics play an important role in determining thefinal consensus state.For instance,the well-studied consensus of multi-agent systems with single-integrator kinematics often converges to a constantfinal value instead.However,consensus for double-integrator dynamics might admit a dynamicfinal value(i.e.,a time function).These important issues motivate the study of consensus under various system dynamics.As a direct extension of the study of the consensus prob-lem for systems with simple dynamics,for example,with single-integrator kinematics or double-integrator dynamics, consensus with general linear dynamics was also studied recently[41]–[43],where research is mainly devoted tofinding feedback control laws such that consensus(in terms of the output states)can be achieved for general linear systems˙x i=Ax i+Bu i,y i=Cx i,(3) where A,B,and C are constant matrices with compatible sizes.Apparently,the well-studied single-integrator kinematics and double-integrator dynamics are special cases of(3)for properly choosing A,B,and C.As a further extension,consensus for complex systems has also been extensively studied.Here,the term consensus for complex systems is used for the study of consensus problem when the system dynamics are nonlinear[44]–[48]or with nonlinear consensus algorithms[49],[50].Examples of the nonlinear system dynamics include:•Nonlinear oscillators[45].The dynamics are often as-sumed to be governed by the Kuramoto equation˙θi=ωi+Kstability.A well-studied consensus algorithm for(1)is given in(2),where it is now assumed that time delay exists.Two types of time delays,communication delay and input delay, have been considered in the munication delay accounts for the time for transmitting information from origin to destination.More precisely,if it takes time T ij for agent i to receive information from agent j,the closed-loop system of(1)using(2)under afixed network topology becomes˙x i(t)=nj=1a ij(t)[x j(t−T ij)−x i(t)].(7)An interpretation of(7)is that at time t,agent i receives information from agent j and uses data x j(t−T ij)instead of x j(t)due to the time delay.Note that agent i can get its own information instantly,therefore,input delay can be considered as the summation of computation time and execution time. More precisely,if the input delay for agent i is given by T p i, then the closed-loop system of(1)using(2)becomes˙x i(t)=nj=1a ij(t)[x j(t−T p i)−x i(t−T p i)].(8)Clearly,(7)refers to the case when only communication delay is considered while(8)refers to the case when only input delay is considered.It should be emphasized that both communication delay and input delay might be time-varying and they might co-exist at the same time.In addition to time delay,it is also important to consider packet drops in exchanging state information.Fortunately, consensus with packet drops can be considered as a special case of consensus with time delay,because re-sending packets after they were dropped can be easily done but just having time delay in the data transmission channels.Thus,the main problem involved in consensus with time delay is to study the effects of time delay on the convergence and performance of consensus,referred to as consensusabil-ity[52].Because time delay might affect the system stability,it is important to study under what conditions consensus can still be guaranteed even if time delay exists.In other words,can onefind conditions on the time delay such that consensus can be achieved?For this purpose,the effect of time delay on the consensusability of(1)using(2)was investigated.When there exists only(constant)input delay,a sufficient condition on the time delay to guarantee consensus under afixed undirected interaction graph is presented in[8].Specifically,an upper bound for the time delay is derived under which consensus can be achieved.This is a well-expected result because time delay normally degrades the system performance gradually but will not destroy the system stability unless the time delay is above a certain threshold.Further studies can be found in, e.g.,[53],[54],which demonstrate that for(1)using(2),the communication delay does not affect the consensusability but the input delay does.In a similar manner,consensus with time delay was studied for systems with different dynamics, where the dynamics(1)are replaced by other more complex ones,such as double-integrator dynamics[55],[56],complex networks[57],[58],rigid bodies[59],[60],and general nonlinear dynamics[61].In summary,the existing study of consensus with time delay mainly focuses on analyzing the stability of consensus algo-rithms with time delay for various types of system dynamics, including linear and nonlinear dynamics.Generally speaking, consensus with time delay for systems with nonlinear dynam-ics is more challenging.For most consensus algorithms with time delays,the main research question is to determine an upper bound of the time delay under which time delay does not affect the consensusability.For communication delay,it is possible to achieve consensus under a relatively large time delay threshold.A notable phenomenon in this case is that thefinal consensus state is constant.Considering both linear and nonlinear system dynamics in consensus,the main tools for stability analysis of the closed-loop systems include matrix theory[53],Lyapunov functions[57],frequency-domain ap-proach[54],passivity[58],and the contraction principle[62]. Although consensus with time delay has been studied extensively,it is often assumed that time delay is either constant or random.However,time delay itself might obey its own dynamics,which possibly depend on the communication distance,total computation load and computation capability, etc.Therefore,it is more suitable to represent the time delay as another system variable to be considered in the study of the consensus problem.In addition,it is also important to consider time delay and other physical constraints simultaneously in the study of the consensus problem.D.QuantizationQuantized consensus has been studied recently with motiva-tion from digital signal processing.Here,quantized consensus refers to consensus when the measurements are digital rather than analog therefore the information received by each agent is not continuous and might have been truncated due to digital finite precision constraints.Roughly speaking,for an analog signal s,a typical quantizer with an accuracy parameterδ, also referred to as quantization step size,is described by Q(s)=q(s,δ),where Q(s)is the quantized signal and q(·,·) is the associated quantization function.For instance[63],a quantizer rounding a signal s to its nearest integer can be expressed as Q(s)=n,if s∈[(n−1/2)δ,(n+1/2)δ],n∈Z, where Z denotes the integer set.Note that the types of quantizers might be different for different systems,hence Q(s) may differ for different systems.Due to the truncation of the signals received,consensus is now considered achieved if the maximal state difference is not larger than the accuracy level associated with the whole system.A notable feature for consensus with quantization is that the time to reach consensus is usuallyfinite.That is,it often takes afinite period of time for all agents’states to converge to an accuracy interval.Accordingly,the main research is to investigate the convergence time associated with the proposed consensus algorithm.Quantized consensus was probablyfirst studied in[63], where a quantized gossip algorithm was proposed and its convergence was analyzed.In particular,the bound of theconvergence time for a complete graph was shown to be poly-nomial in the network size.In[64],coding/decoding strate-gies were introduced to the quantized consensus algorithms, where it was shown that the convergence rate depends on the accuracy of the quantization but not the coding/decoding schemes.In[65],quantized consensus was studied via the gossip algorithm,with both lower and upper bounds of the expected convergence time in the worst case derived in terms of the principle submatrices of the Laplacian matrix.Further results regarding quantized consensus were reported in[66]–[68],where the main research was also on the convergence time for various proposed quantized consensus algorithms as well as the quantization effects on the convergence time.It is intuitively reasonable that the convergence time depends on both the quantization level and the network topology.It is then natural to ask if and how the quantization methods affect the convergence time.This is an important measure of the robustness of a quantized consensus algorithm(with respect to the quantization method).Note that it is interesting but also more challenging to study consensus for general linear/nonlinear systems with quantiza-tion.Because the difference between the truncated signal and the original signal is bounded,consensus with quantization can be considered as a special case of one without quantization when there exist bounded disturbances.Therefore,if consensus can be achieved for a group of vehicles in the absence of quantization,it might be intuitively correct to say that the differences among the states of all vehicles will be bounded if the quantization precision is small enough.However,it is still an open question to rigorously describe the quantization effects on consensus with general linear/nonlinear systems.E.RemarksIn summary,the existing research on the consensus problem has covered a number of physical properties for practical systems and control performance analysis.However,the study of the consensus problem covering multiple physical properties and/or control performance analysis has been largely ignored. In other words,two or more problems discussed in the above subsections might need to be taken into consideration simul-taneously when studying the consensus problem.In addition, consensus algorithms normally guarantee the agreement of a team of agents on some common states without taking group formation into consideration.To reflect many practical applications where a group of agents are normally required to form some preferred geometric structure,it is desirable to consider a task-oriented formation control problem for a group of mobile agents,which motivates the study of formation control presented in the next section.IV.F ORMATION C ONTROLCompared with the consensus problem where thefinal states of all agents typically reach a singleton,thefinal states of all agents can be more diversified under the formation control scenario.Indeed,formation control is more desirable in many practical applications such as formationflying,co-operative transportation,sensor networks,as well as combat intelligence,surveillance,and reconnaissance.In addition,theperformance of a team of agents working cooperatively oftenexceeds the simple integration of the performances of all individual agents.For its broad applications and advantages,formation control has been a very active research subject inthe control systems community,where a certain geometric pattern is aimed to form with or without a group reference.More precisely,the main objective of formation control is to coordinate a group of agents such that they can achievesome desired formation so that some tasks can befinished bythe collaboration of the agents.Generally speaking,formation control can be categorized according to the group reference.Formation control without a group reference,called formationproducing,refers to the algorithm design for a group of agents to reach some pre-desired geometric pattern in the absenceof a group reference,which can also be considered as the control objective.Formation control with a group reference,called formation tracking,refers to the same task but followingthe predesignated group reference.Due to the existence of the group reference,formation tracking is usually much morechallenging than formation producing and control algorithmsfor the latter might not be useful for the former.As of today, there are still many open questions in solving the formationtracking problem.The following part of the section reviews and discussesrecent research results and progress in formation control, including formation producing and formation tracking,mainlyaccomplished after2006.Several milestone results prior to 2006can be found in[69]–[71].A.Formation ProducingThe existing work in formation control aims at analyzingthe formation behavior under certain control laws,along with stability analysis.1)Matrix Theory Approach:Due to the nature of multi-agent systems,matrix theory has been frequently used in thestability analysis of their distributed coordination.Note that consensus input to each agent(see e.g.,(2))isessentially a weighted average of the differences between the states of the agent’s neighbors and its own.As an extensionof the consensus algorithms,some coupling matrices wereintroduced here to offset the corresponding control inputs by some angles[72],[73].For example,given(1),the controlinput(2)is revised as u i(t)= n j=1a ij(t)C[x j(t)−x i(t)], where C is a coupling matrix with compatible size.If x i∈R3, then C can be viewed as the3-D rotational matrix.The mainidea behind the revised algorithm is that the original controlinput for reaching consensus is now rotated by some angles. The closed-loop system can be expressed in a vector form, whose stability can be determined by studying the distribution of the eigenvalues of a certain transfer matrix.Main research work was conducted in[72],[73]to analyze the collective motions for systems with single-integrator kinematics and double-integrator dynamics,where the network topology,the damping gain,and C were shown to affect the collective motions.Analogously,the collective motions for a team of nonlinear self-propelling agents were shown to be affected by。
Two-dimensional Quantum Field Theory, examples and applications

Abstract The main principles of two-dimensional quantum field theories, in particular two-dimensional QCD and gravity are reviewed. We study non-perturbative aspects of these theories which make them particularly valuable for testing ideas of four-dimensional quantum field theory. The dynamics of confinement and theta vacuum are explained by using the non-perturbative methods developed in two dimensions. We describe in detail how the effective action of string theory in non-critical dimensions can be represented by Liouville gravity. By comparing the helicity amplitudes in four-dimensional QCD to those of integrable self-dual Yang-Mills theory, we extract a four dimensional version of two dimensional integrability.
2 48 49 52 54 56
5 Four-dimensional analogies and consequences 6 Conclusions and Final Remarks
纳撒尼尔·霍桑作品《拉帕齐尼的女儿》与圣经的联系

纳撒尼尔·霍桑作品《拉帕齐尼的女儿》与圣经的联系发布时间:2021-04-22T10:36:23.800Z 来源:《教育学文摘》2021年第3期作者:卿瑜[导读] 纳撒尼尔·霍桑是十九世纪美国文学史上具有代表性的浪漫主义小说家卿瑜重庆工业职业技术学院ABSTRACT: Nathaniel Hawthorne was a representative romantic writer in the 19th century American literature, who was praised as “the first great novelist of American nation.” He was greatly affected by Calvinism believed that the “ Original Sin” was the source of evil. In his story Rappaccini’s Daughter, he exquisitely displayed his idea about evil in human nature. Also, many Biblical Similarities can be found in the story. The purpose of this paper is to introduce, compare, and discuss the story in order to find its close ties with the Holy Bible.KEY WORDS: Nathaniel Hawthorne, Original Sin, evil, Holy Bible摘要: 纳撒尼尔·霍桑是十九世纪美国文学史上具有代表性的浪漫主义小说家。
人们称赞他为“美国第一位伟大的小说家。
” 霍桑深受加尔文主义的影响认为“原罪”是人类恶性的根源。
硕士研究生入学考试大纲-863英语语言学及英汉互译

全国硕士研究生入学统一考试英语语言学及英汉互译考试大纲一、考试要求掌握普通语言学的基本概念、基础理论,并能运用理论进行简单的语言结构分析。
有较扎实的语言功底,较强的英汉书面表达能力,掌握并灵活运用常用英汉翻译技巧。
二、考试内容1、普通语言学的定义及主要分支;2、语言的性质:语言、语言的特征、语言的功能;3、语音学(phonetics)中的基本术语;4、语素、词缀及主要构词法;5、句法结构分析;6、语义关系及语义成分分析;7、言语行为理论和会话涵义理论;8、现代语言学的主要理论和流派;9、英译汉;10、汉译英。
三、题型Section A1、术语解释(20分:10题)2、填空(20分:20题)3、简答(30分:6题)4、详答(20分:1题)Section B1、英译汉(30分:一个约250单词的段落)2、汉译英(30分:一个约250字的段落)四、参考书胡壮麟主编.《语言学教程》(修订版).北京大学出版社,2002年冯庆华.《实用翻译教程》(第一版).上海外语教育出版社,2002年五、题型示例及参考答案Section APart I. Define the following terms briefly (20 points, 2 points each).1. Displacement2. MetalanguagePart II. Fill the blanks with proper words (20 points , 1 point each blank).1. By _____ is meant the property of having two levels of structures, such that units of the primary level are composed of elements of the secondary level and each of the two levels has its own principles of organization.2.____ studies the rules governing the structure , distribution, and sequencing of speech sounds and the shape of syllables.Part III. Answer the following questions briefly(30 points, 4 points each).1.Do you think that onomatopoeia indicates a non-arbitrary relationship between form and meaning?2. To what extent is phonology related to phonetics and how do they differ?Part IV. Give a detailed description of the special features of American structuralism(20 points). Section BPart I. E-C Translation (30 points)What social morality and social conscience leaves out is the narrower but very significant concept of honor------as opposed to what is sometimes called merely “socially desirable conduct.” The man of honor is not content to ask merely whether this or that will hurt society, or whether it is what most people would permit themselves to do. He asks, and he asks first of all, would it hurt him and his selfrespect? Would it hishonor him personally? It was a favorite and no doubt sound argument among early twentieth-century reformers that “playing the game” as the gentleman was supposed to play it was not enough to make a decent society. They were right: it is not enough. But the time has come to add that it is nevertheless indispensable. I hold that it is indeed inevitable that the so-called social conscience unsupported by the concept of personal honor will create a corrupt society. But suppose that it doesn’t. Suppose that no one except the individual suffers from the fact that he sees nothing wrong in doing what everybody else does. Even so, I still insist thatfor the individual himself nothing is more important than this personal, interior sense of right and wrong and his determination to follow that rather than to be guided by that everybody does or merely the criterion of “social usefulness.”It is impossible for me to imagine a good society composed of men without honor.Part II. C-E Translation (30 points)他在父亲的教导下“发愤用功”,其实他读书还是出于喜好,只似馋嘴佬贪吃美食:食肠很大,不择精粗,甜咸杂进。
超对称理论导论

• Discrete symmetries: C, P, and T.
dimensional supersymmetry algebra is given in the Appendix; here we will be content with checking some of the features of this algebra. The anticommutator of the QA α with their adjoints is: { QA ˙ } α , QβB =
,
A where the CB are complex Lorentz scalar coefficients. Taking the adjoint of the left-hand side of Eq. 2, using m σα ˙ β † m = σβ α ˙
, , (3)
† QA α
= Qα ˙
A
A tells us that CB is a hermitian matrix. Furthermore, since {Q, Q} is a positive A definite operator, CB is a positive definite hermitian matrix. This means that A A we can always choose a basis for the QA α such that CB is proportional to δB . The factor of two in Eq. 2 is simply a convention. The SUSY generators QA α commute with the translation generators:
IEEE 519-1992 谐波分析指南说明书

Harmonic Analysis and IEEE 1992 GuidelinesIntroductionIEEE 519-1992 provides guidelines for applying limits to the level of harmonic distortion that a utilitycustomer may inject into the power system. This is a concern, since Adjustable Frequency Drives (AFDs) can contribute significant harmonic distortion to a power system. The guidelines pertain to percentharmonic current and voltage distortion at the point of common coupling (PCC), which is defined as the point where the utility connects to multiple customers.Although many customers and system designers interpret the PCC to be at the AFD input or various locations within the 480V distribution, this is not consistent with the intent of IEEE guidelines. There are no limits recommended for individual loads, only for the overall system. Customers and system designers can choose the point of analysis (POA) where they desire, but it may add substantial filtering costs if the POA is downstream of the PCC.Current distortion drawn through an impedance (transformer, cable resistance) causes voltagedistortion.The distorted current will also cause additional heating of the input cables and the transformer. Excessive voltage distortion is a concern, since it may cause interference with other electronic equipment and additional motor heating.IEEE 519-1992 recommends different limits on Individual Harmonics (I h ) and Total Demand Distortion (TDD), depending on the I SC /I L ratio. I SC is the short circuit current at the PCC, and I L is the maximum demand load current (fundamental) at the PCC. More current distortion is allowed at higher I SC /I L ratios, since voltage distortion decreases as the ratio increases.The voltage distortion guidelines for IEEE-1992 (at 480V) remain the same as IEEE 519-1981:∙ 3% — Special systems (i.e. hospitals or universities) ∙ 5% — General systems∙ 10% —Dedicated systems (AFDs only)Application Note AP04014002EHarmonic Analysis and IEEE 1992 GuidelinesEffective July 20142 EATON CORPORATION The best way to estimate AFD harmonic contribution to an electrical system is to perform a harmonicanalysis based on known system characteristics. An individual AFD may meet the IEEE guidelines in one system and not meet the guidelines in another system depending on the pre-existing characteristics of the specific system.Some AFD vendors, upon seeing a specification requirement for IEEE 519-1992, will simply add a line reactor. This is the wrong approach, since some systems will not require a line reactor and others will not benefit sufficiently to meet the guidelines (or the specification).For a free computerized harmonic analysis of AFD contribution to system harmonics or for additional information, contact your local Eaton sales office. A one-line drawing of the electrical distribution system and specification criteria will be required. A harmonic analysis worksheet for required data is attached.Any additional harmonic mitigation equipment requirements will be determined during the analysis. If there are harmonic constraints during AFD operation on a standby generator, a separate analysis will be required for the generator, and assumptions on load-shedding strategies during generator operationshould be provided. Several data runs may be required to evaluate various harmonic mitigation methods. Resultant recommendations may include 1%, 3%, or 5% line reactors, phase-shifting transformers, filters, or CPX9000 Clean Power.Helpful Facts∙ Harmonics are supply system dependent. As the short circuit amps available (SCA) increase, %voltage distortion decreases, and % current distortion increases.∙ For each PCC or POA analysis required, provide SCA and IL (load current) values for that point. SCAs used must be without motor contribution to SCA.∙Current distortion percentages are dependent on overall system loading. As linear loads (non-harmonic loads such as AC motors on line power) increase, the percent current distortion decreases through dilution.∙ % current distortion is the same across a transformer. Voltage distortion percentage is lower on the primary than on the secondary, assuming the harmonic loads are on the secondary. ∙A harmonic analysis is only as accurate as the assumptions made for the analysis!Harmonic Analysis Data Worksheet(Use separate sheets if necessary. Provide a 1-line drawing or sketch.)lated voltage distortion and higher calculated current distortion on the transformer secondary.Distribution Transformer(s) Data:Generator Data:Describe load-shedding scheme for generator operation in “AFD Data” below.AFD Data:kVAImpedanceX/R Ratio#1#2kW kVAVoltsX"dI L (amps)#1#2AFD hpType (SVX9000,Clean Power, etc.)Quantity Operated on Line / GeneratorDesired, existing or specified line reactor or isolation transformer (none, 1% / 3% / 5%)//////Application Note AP04014002E Effective July 2014Harmonic Analysis and IEEE 1992 Guidelines3 EATON CORPORATION Application Note AP04014002E Harmonic Analysis and IEEE 1992 Guidelines Effective July 2014Additional HelpIn the US or Canada: please contact the Technical Resource Center at 1-877-ETN-CAREor 1-877-326-2273 option 2, option 6.All other supporting documentation is located on the Eaton web site at /DrivesEaton1000 Eaton BoulevardCleveland, OH 44122 USA© 2014 EatonAll Rights ReservedPrinted in USAPublication No.AP04014002EJuly 2014Eaton is a registered trademarkof Eaton Corporation.All other trademarks are propertyof their respective owners。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
a rXiv:h ep-th/959165v128Se p1995TAUP-2291-95Sept,1995(T)Duality and other Exotic Gauge Dynamics in Softly Broken Supersymmetric QCD Ofer Aharony 1,2,3,Jacob Sonnenschein 2,3School of Physics and Astronomy Beverly and Raymond Sackler Faculty of Exact Sciences Tel Aviv University,Ramat Aviv,Tel Aviv 69978,Israel Michael E.Peskin 4Stanford Linear Accelerator Center Stanford University,Stanford,California 94309USA and Shimon Yankielowicz 3,5CERN,Geneva,Switzerland ABSTRACT We analyze the theory of softly broken supersymmetric QCD .Exotic be-havior like spontaneously broken baryon number,massless composite fermions and Seiberg’s duality seems to persist also in the presence of (small)soft super-symmetry breaking.We argue that certain,specially tailored,lattice simulationsmay be able to detect the novel phenomena.Most of the exotic behavior does not survive the decoupling limit of large SUSY breaking parameters.Based on a talk presented by J.S in SUSY 95.1Introduction and summary*(1)We study multi-flavored four dimensional QCD by adding soft supersymmetry breaking (SSB)terms to supersymmetric QCD(SQCD)*(2)Certain exotic phenomena discovered in SQCD persist also in the regime of small, compared toΛQCD,SSB parameters.*(3)The exotic behavior includes(i)a baryon number violating vacuum,(ii)massless composite fermions and(iii)Seiberg’s duality[1].*(4)In the decoupling limit most of the“exotic”phenomena disappear.*(5)We argue that all the exotic behavior of the region close to the SUSY limit should be detected in lattice simulations.2On the effective action of SQCDSU(N c)SQCD contains a gauge superfield Wα,a chiral quark and an anti quark superfields Q i a,SU(N c)SU(N f)R U(1)RN c1N cQ N c−1(N f−N c)/N fWα10Q j.(2.2) Beginning at N f=N c,S eff is built also from chiral baryon and anti-baryon superfields.The baryonB i1···i(N f−N c)=ǫa1···a N cǫj1···j N c i1···i(N f−N c)Q j1a1···Q j N c aN c,(2.3)transforms in the(N f−N c)-index antisymmetric tensor representation of SU(N f)L,and, similarly,an antibaryon chiral superfield Q.Using the gauge supermultiplet,it is possible to build the(massive)“glue ball”chiral superfieldS=−tr[WαWα]=tr[λ·λ]+···.(2.4) The K¨a hler potential which determines the kinetic energy terms of thefields T,B,andB]=A T tr[T†T]+A B(B†B+B).(2.5) Our main results will rely on weaker assumptions about the K¨a hler potential,in particular, that it is nonsingular on the space of supersymmetric vacuum states.However,we will support our general remarks by explicit calculations using this simple model.We expect (2.5)to be the correct form of the K¨a hler potential near the origin of moduli space,in the cases for which the mesons and baryons give an effective infrared description of the theory. This is not the case for N c>N f[5].2.1Soft Supersymmetry BreakingWe break supersymmetry by adding mass terms for the squarks and gauginofields,∆L=−m2Q |Q|2+|Q,and S are the scalar componentfields of the superfields.The squark mass term is the unique soft supersymmetry breaking term which does not break any of the global symmetries(2.1)of the original model.The gaugino mass term breaks only the U(1)R symmetry,and thus breaks the global symmetry of the supersymmetric model down to that of ordinary QCD with N f masslessflavors.Actually,there are two consistent possibilities for SSB theories which we denote by R and RR:m2Q=0m g=0=⇒m2Q →∞standard QCD with N f quarks+adjoint fermionsR:m2Q=0m g=0=⇒m2Q ,m g→∞standard QCD with N f quarksSince we will be working in the language of the low-energy effective Lagrangian,we must ask how the supersymmetry breaking term(2.6)shows up in this Lagrangian.To work this out,rewrite(2.6)in the superfield form∆L= d4θM Q(Q†e V Q+Q)+ d2θM g S+h.c.(2.7)where M Q is a vector superfield whose D component equals(−m2Q)and M g is a chiral superfield whose F component equals m g.It is straightforward to see that these superfields are gauge-invariant and neutral under all of the global symmetries.The effective Lagrangian description of∆L for N f≤N c+1is then given by writing the most general Lagrangian built from T,B,M g.The supersymmetry breaking terms have an ambiguity related to that of the K¨a hler potential,because many possible invariant structures can be built from T B,andB†M g M(T,B,B)is a function of the effective Lagrangian superfields which is neutral under the global symmetries.(ii)The quantity S in(2.8)should be a combination of the effective Lagrangian chiral superfields which has the quantum numbers of S.In general,this condition restricts that function to be proportional to the expectation value of S as determined from the effective Lagrangian of refs.[6,7]which includes S as a basicfield.(iii)The squark mass terms in(2.8)are not the most general terms that can be written down.Higher order terms in thefields,suppressed by powers ofΛ,may appear.However, we expect(2.8)to be approximately true near the origin of moduli space T=B=B T which the reader might take as qualitative guidance.2(vi)It is important for our analysis that the behavior of the theory is non–singular when adding the squark and gluino masses,i.e.that no new non–perturbative effects occur. In general it is not possible to prove this in non–supersymmetric theories,but a proof of this is possible in softly broken supersymmetric theories,when the soft breaking can be viewed as arising via spontaneous breaking of supersymmetry.[8]3Baryon number violation(N f=N c)The cases of N c>N f are discussed in ref.[2],here we start with the case N f=N c.The low-energy effective Lagrangian of the supersymmetric limit contains both meson and baryon superfields.The quantum numbers of the T i j,B andSU(N f)L U(1)BT i j N f N f0B11B1−1B=1.(3.9) Several forms for the superpotential are consistent with this relation.The S-dependent superpotential,for example,has the form W=S log(det T−BB obeying the constraint(3.9),for which the vacuum energy vanishes in the supersymmetric limit,and study the behavior of the supersymmetry breaking potential over this space.For the R models,the soft supersymmetry breaking terms(2.8)lead to the potential∆V=B T m2Q tr[T†T]+B B m2Q(B†B+B).(3.10) Using SU(N f)×SU(N f),we can diagonalize T to complex eigenvalues t i.Parameterize thebaryonfields as B=xb,x b with x and b complex.This potential has three types ofstationary pointsւ⇓ցb=0∀i|t i|=1T=0,b=±1for B B>(N f/2)B Tglobally stablewith x=1in all cases.The method of effective Lagrangians cannot tell us which of the two vacuum states at b=0and T=0is the preferred one.This depends on the ratio B B/B T, which is a phenomenological input to the effective Lagrangian analysis.The vacuum at T=0is locally stable if B B>B T and is globally stable if B B>(N f/2)B T.Our naive estimate puts the theory just at the boundary at which the two vacuum states have equal energy.The structure of the spectrum in the b=0and T=0cases is summarized as follows (for details see[2]).T=0vacuum SU(N f)D×U(1)B×U(1)RB=b+c,B=(1+b+c),t V+it A=− N f(b2−c2)2det T+1t AI-NG bosons t A massivem2V i=2m2T=B T m2−=2m2cR=B Bm2+=2fermionsB =0B=0Notice that since(3.9)is a superfield constraint,it also removes one fermion from the theory,specifically,the fermionic partner of det[T]or of B+B are all invariant under U(1)R,while a superpotential has R charge2,this term does not contribute to the superpotential to any order in m g.There are possible K¨a hler potential terms involving M g.However,near the vacuum with b=0,these will be polynomials in B andM g(C T det T+C B BB)+M g S)+h.c.,(3.12) and integrates out S,onefinds a breaking term∆L= d4θB)+h.c.,(3.13)which gives qualitatively similar results.In the following discussion,we will work with(3.11). Once the U(1)R is broken it does not protect fermions from acquring mass.Around b=0 theflavor adjoint and baryonic fermions,acquire the following masses mψi=1A Tm g and mψB=C BA Bm g.3.1Toward the Decoupling LimitThe transition from the region of weak supersymmetry breaking to the decoupling limit m2Q,m g→∞is very different in the four models of R and R with vacua at b=0and T=0.(i)R b=0-In this case(like in the cases of N c>N f)the global group SU(N f)×SU(N f)×U(1)B is broken spontaneously to SU(N f)×U(1)B,leaving no massless parti-cles except for the required Goldstone bosons.It is reasonable to expect that there is a smooth transition from the situation of weak supersymmetry breaking to the decoupling limit m2Q,m g→∞.In QCD,chiral symmetry breaking is characterized by a nonzero vac-uum expectation value of the quark-antiquark bilinear,ψi Qψ2N c−N f Q .Thus,the F term of T does obtain an expectation value inthe vacuum state that we have found.This expectation value naturally becomes a nonzero expectation value for the quark bilinear in the decoupling limit.As m Q increases,the quark bilinear becomes larger while the squark bilinear becomes smaller,in exact accord with our expectations.(ii)R b=0-As m2Q is taken to infinity,the squarks decouple,and the model becomes a purely fermionic QCD theory with N f quarkflavors plus one fermionflavor in the adjoint representation of the gauge group.For small values of the supersymmetry breaking mass m2Q,this vacuum contains massless fermions corresponding to the fermionic components of the superfields T,B,and2.But it is also possible to buildcomposite bound state with the same quantum numbers purely out of fermions,by replacingQ i→λαψi Qα,Qαj,(3.14) whereαis a two-component spinor index and the gauge indices are implicit.Notice that this combination has the same quantum numbers as the squark,including zero R charge. Then,for example,the fermion created by T i j could be constructed asψTαi j→ψi Qαλβψof the condensate λ·λ spontaneously breaks U(1)R.In this case,the physics would revert to the usual symmetry-breaking pattern of QCD,and the composite fermions would become massive.The gluino condensate would make itself felt only by providing an extra SU(N f)-singlet Goldstone boson.We will refer to this scenario as‘option2’.(iii)R T=0-In this model,in the supersymmetric limit,the massless composite fermions belong to the(N f,Qj ,and gauge-invariant states must contain an even number of these.For N c odd,however,it is possible to build composites with the correct quantum numbers,as follows:ψTαi j→ǫab···dψi Qαaǫjk···ℓQb···Q˙γd,(3.16)whereQis the right-handed fermionfield in(N f,−1)+(1,1,−1)of the unbroken symmetry group.corresponding to the fermions in T and a linear combination of the fermions in B and4NoχSB,massless fermions(N f=N c+1)In the case of N f=N c+1,the low-energy effective Lagrangian in the supersymmetric limit is expressed in terms of the baryon,anti-baryon and meson superfields which transform under the global symmetry as follows(2.1)B:(N f N f T:(N f,N f(4.17)where the second subscript is the R charge of the scalar component of the superfield.In the supersymmetric theory the low energy effective theory is described(at least near the origin of moduli space)by the K¨a hler potential given by(2.5),and by the following superpotential [13]:W=B i T i jB i=01B j N f=0.(4.19)As was argued in[13],these equations correctly describe the moduli space of vacuum states in the full quantum theory.At the origin of the moduli space,<T>=<B>=<B indicated in(2.8).Since we are adding terms to the potential which are positive and vanish at the origin of moduli space,it is obvious that the origin becomes the only vacuum state of the theory.All of the scalar particles in the effective theory obtain mass terms proportional to B T m2Q or B B m2Q.Though all of the scalars obtain mass,all of the fermions remain massless.The su-perpotential(4.18)is a least cubic in thefields,thus,any mass term derived from this superpotential vanishes at the origin.Similarly,in the R case,the M g term in(2.8)requires a function of T,B,andB†B is needed to satisfy the‘t Hooft anomaly conditions for the global symmetry group SU(N f)×SU(N f)×U(1)B×U(1)R. 5Seiberg’s duality in SSB models(N f≥N c+2)No solution of the’t Hooft anomaly matching conditions for SQCD involving gauge invariant bound states is known for N f>(N c+1).However,Seiberg has suggested a compellingsolution to these constraints in terms of a novel type of duality in which new gauge degrees of freedom are dual to the original quarks and gluons[1].The pair of dual theories are “Electric theory”SU(N c)gauge theoryˆa=1,...,(N f−N c)Q i a,(N f,1,1qˆa i,(,N c/N f)N f−N c,N c/N f)N f,−1N f−N cN f,0,2(N f−N c)/N f)W=0q jˆaOn top of the fact that these theories have the same’t Hooft anomalies,they were shown to be equivalent in the infrared also by analyzing their“chiral rings”,flat directions and mass perturbations.In the supersymmetric limit of the magnetic theory the scalar potential has a moduli space of vacua,which includes the point<T>=<q>=<q|2),(5.20) at least near the origin of moduli space.After we add this perturbation,the only minimum of the potential is at<T>=<q>=<electric theory AS free*:IR free9N c*IR*117N cfixed9magnetic theory IR free:*AS freeThe picture describes a scenario where in the range between the:signs,namely112N c for the R case and between the∗signs,namely,92N c for the R case,the two theoriesflow to a commonfixed point in the IR.In the range N f<117N c)the R(R)systemflows to the free magnetic theory in the IR,whereas for N f>112N c)the picture in the infrared for R(R)is that of the free electric theory.The discussion of the decoupling limit for these theories is very similar to that for the N f=N c theory.Thus,for those values of N f which have massless fermions for small values of m Q we must have a second-order phase transition as m Q is increased.It is not clear how the theory behaves on the other side of this phase transition.In the R case obviously only a SU(N f)×U(1)B symmetry remains,with no massless fermions.This situation is schematically described as followsR⇓SU(N f)×U(1)B SU(N f)×U(1)B×U(1)Rmassive fermions(iii)To analyze this question,consider the renormalization group equations for an approximately supersymmetric gauge theory.Viewed as a conventional renormalizable gauge theory,SQCD has three coupling constants,the gauge coupling g,the quark-squark-gluino coupling gλ,and the four-scalar coupling g D.The scalar potential has the specific formV=g2DQτAQ†.The most general linear combination of these invariants can be generated by the renormalization groupflow.We can view the effects of renormalization relatively simply,however,by restricting our attention to the vicinity of the surface given by the three couplings g,gλ,and g D.In this surface,the beta functions of the three couplings are given byβg=−1(4π)2gλg2(3N c+3C2(N c))−g3λ(3C2(N c)+N f)βg2D =−12Q†{τA,τB}Q+Q† 2.(6.22)On the surface g2E=0,the beta function for g2E isβg2E =−1With this provision to avoid possiblefirst-order phase transitions,we expect that lattice simulations with an approximately supersymmetric action can reach the continuum limit and test our predictions for softly broken supersymmetric QCD.ACKNOWLEDGEMENTSWe are grateful to Eliezer Rabinovici and the organizers of the1995Jerusalem Winter School for bringing us together.We thank Nathan Seiberg for a stimulating set of lectures at the school which ignited our interest in this problem and for several useful discussions.We thank Shmuel Nussinov and Gabrielle Veneziano for stimulating conversations,and we are especially grateful to Tom Banks for emphasizing the importance of QCD inequalities.MEP thanks the members of the high energy group for their hospitality at Tel Aviv University during the initial phase of this work.References[1]N.Seiberg,Nucl.Phys.B435,129(1995).[2]O.Aharony,M. E.Peskin,J.Sonnenschein and S.Yankielowicz“Exotic Non-Supersymmetric Gauge Dynamics from Supersymmetric QCD”hep-th/9507013,to ap-pear in Phys.Rev.D,.([3]MV A.Masiero and G.Veneziano,Nucl.Phys.B249,593(1985).[4]A.Masiero,R.Pettorino,M.Roncadelli,and G.Veneziano,Nucl.Phys.B261,633(1985).[5]See footnote in ref.[2][6]G.Veneziano and S.Yankielowicz,Phys.Lett.113B,321(1982).[7]T.Taylor,G.Veneziano,and S.Yankielowicz,Nucl.Phys.B218,493(1983).[8]N.Evans,S.D.H.Hsu and M.Schwetz,“Exact Results in Softly Broken Supersym-metric Models”,YCTP-P8-95,hep-th/9503186[9]I.Affleck,M.Dine,and N.Seiberg,Phys.Rev.Lett.51,1026(1983).[10]D.Weingarten,Phys.Rev.D51,1830(1983).[11]C.Vafa and E.Witten,Nucl.Phys.B234,173(1984).[12]K.Intriligator and N.Seiberg,“Duality,Monopoles,Dyons,Confinement and ObliqueConfinement in Supersymmetric SO(N c)Gauge Theories”,RU-95-3,hep-th/9503179.[13]N.Seiberg,Phys.Rev.D49,6857(1994).[14]S.Coleman and E.Weinberg,Phys.Rev.D7,1888(1973).。
