ISOMORPHISMS AND DERIVATIONS IN C*-ALGEBRAS
笛卡尔的本体论之争

笛卡尔的本体论之争首先周一公布2001年6月18日;实质性修改太阳2006年10月15日笛卡尔的本体论(或先验)的论点,既是哲学的一个最迷人,他的理解方面的不足。
论据与魅力源于努力证明神的存在,从简单的处所,但功能强大。
存在是产生立即从清晰和明确的想法是一个无比完美。
讽刺的是,简单的说法也产生了一些误读,加剧了部分由笛卡尔没有一套单一版本。
该声明的论点主要出现在第五沉思。
这种说法因果来得早在接踵而至的一个神的存在,沉思在第三,不同的证据提出问题的两项之间的秩序和关系。
重复笛卡尔哲学原理,包括本体论争论的几个文本等中央。
他还辩解首先由一些主要的知识分子,他在一天,严厉打击反对第二次回复,和第五。
笛卡尔不是第一位哲学家,制订一个本体论的论点。
一个早期版本的说法已大力安瑟伦辩护圣在11世纪,然后圣托马斯阿奎那批评由当代),后来被命名为Gaunilo和尚(安瑟伦(尽管他的言论是针对然而,另一个版本参数)。
阿奎那的批评被视为如此具有破坏性,本体论的争论了数百年死亡。
它的出现,作为一个同时代的惊喜笛卡尔,他应该试图复活它。
虽然他声称没有被证明的熟悉安瑟伦的版本,笛卡尔似乎他自己的工艺参数,以阻止传统的反对。
尽管相似之处,笛卡尔的论点的版本不同于安瑟伦方式在重要的。
后者的版本被认为要从定义这个词的含义“上帝”,上帝是一个被一大于不能设想。
笛卡尔的观点相反,中,主要是基于两个他的哲学的中心原则-天生的思想理论和学说明确的印象和独特的。
他声称不依赖于上帝的任意定义,而是一种天生的想法,其内容是“的。
” 笛卡尔的版本也非常简单。
神的存在是直接从推断的事实,有必要存在的想法是包含在一个清晰而鲜明的超级完美的存在。
事实上,在一些场合,他建议,所谓的本体论“的论调”是不是一个正式的哲学偏见的证据,而是在所有不言而喻的公理直观地掌握了一个心灵的自由。
笛卡尔的本体论的争论相比往往以几何论证,认为有必要存在的想法不能排除再从神比事实平等的角度,其角度,例如两权,可以被排除在一个三角形的想法。
The_Shengsheng_Philosophy_of_the_Book_of_Changes_a

Abstract: As far as its origin goes, the concept of sheng (production) or shengsheng (production–reproduction) was inherently emphasized in ancient theories of cosmogenesis informed by the Heavenly Canopy theory. From the beginning, the three versions of the Changes reflected a binary mode of thinking. The Commentaries on the Book of Changes highlight the shengsheng philosophy which consists in roughly three points: (1) the binary opposition between Heaven (higher, yang, honorable) and Earth (lower, yin, humble) constitutes the genetic structure in which the shengsheng philosophy was conceived; (2) the concept of shengsheng involves two principles, that is, “change” and “production and completion,” which are also where its value and purpose lie; and (3) the shengsheng philosophy is characterized by continuation, connection, and mutual interaction. The shengsheng philosophy can be interpreted through the threefold meaning of yi in the Commentaries on the Book of Changes, that is, simplicity, change, and changelessness. As regards this proposition, “Qian Opens the Channel” and Zheng Xuan o ered cosmogenetic interpretations, while Kong Yingda proposed one based on the substance–function theory. According to Neo-Confucians, sheng or shengsheng is the very law (principle) of the cosmos, that is, “the mind of Heaven and Earth,” and even the substance of the cosmos itself. Keywords: shengsheng, Book of Changes, Commentaries on the Book of Changes, Kong Yingda
deterrence

Conflict and Deterrence under Strategic Risk∗Sylvain Chassang Princeton University chassang@Gerard Padr´o i Miquel London School of Economics and NBERg.padro@January2010AbstractWe examine the determinants of cooperation and the effectiveness of deterrence when fear is a motive for conflict.We contrast results obtained in a complete informa-tion setting,where coordination is easy,to those obtained in a setting with strategicrisk,where players have different information about their environment.These twostrategic settings allow us to identify and distinguish the role of predatory and pre-emptive incentives as determinants of cooperation and conflict.We show that whileweapons unambiguously facilitate peace under complete information,this does nothold anymore under strategic risk.Rather,wefind that increases in weapon stockscan have a non-monotonic effect on the sustainability of cooperation.We also showthat under strategic risk,inequality in military strength can actually facilitate peaceand that anticipated peace-keeping interventions may improve incentives for peacefulbehavior.Keywords:cooperation,deterrence,strategic risk,global games,conflict,interven-tion,exit games.JEL classification codes:D74,C72,C73∗We are grateful to Sandeep Baliga,Micael Castanheira,Jon Eguia,Joan Esteban,Christian Hellwig, Matt Jackson,Patrick Legros,David Miller,Andrea Prat,Jesse Shapiro,Joel Sobel and Flavio Toxvaerd for comments and conversations.The paper also benefited from feedback by seminar participants at Sussex University,Universit´e Libre de Bruxelles,Rochester,UC Berkeley,UCSD,Cambridge University,and con-ference participants at the2008AEA meetings,the Polarization and Conflict Group Meeting at LSE,the UC Berkeley Conference on Endogenous Institutions and Conflict,as well as the ESSET meeting at Gerzensee. Andrew Robinson provided excellent research assistance.All remaining errors are,of course,our own.11IntroductionThe usual rationale for deterrence is closely related to the rationale behind grim trigger pun-ishment in a repeated prisoners’dilemma.Imagine two neighboring groups that repeatedly decide whether to be peaceful–i.e.to cooperate–or to launch a surprise attack on each other.A peaceful equilibrium can only be sustained if the short-run gains from a surprise attack are counterbalanced by the long-run costs of triggering conflict.In this context,if both groups accumulate weapons,the cost of conflict increases,thereby improving incen-tives for peaceful behavior.This is the logic of deterrence,which reflects the idea frequently highlighted in the literature on repeated games that harsher punishments should improve incentives for cooperation.1The symmetric accumulation of weapons,insofar as it generates higher costs of war,should facilitate peace.This paper examines the limits of this argument by contrasting the mechanics of cooper-ation and deterrence under complete information and under strategic risk,i.e.when players do not share a common understanding of their environment.While the complete information model suggests unambiguous predictions about the effect of weapons on peace,and about the impact of inequality on cooperation,these predictions need to be considerably nuanced once strategic risk is taken into account.We develop these points in detail and emphasize the importance of both predatory and preemptive incentives in determining the sustainability of cooperation under strategic risk.We model conflict as a very stylized dynamic exit game,keeping grim-trigger strategies in a repeated game as a benchmark.In each period,players decide whether to be peaceful or attack.When both players choose to be peaceful,they enjoy the economic benefits of peace and the game moves on to the next period.However,if one of the players attacks, conflict begins and players obtain exogenous continuation values.2Our model of strategic 1See for instance Abreu(1988)on penal codes.Garfinkel(1990)makes a similar point in the context of conflict and armament.2Because the players’payoffs upon conflict are exogenously specified,this game is not a repeated game. However,trigger strategies of a repeated game are naturally mapped into an exit game in which continuation values upon conflict are those that players obtain from repeatedly playing(Attack,Attack).Therefore,this2risk follows the global games literature.3More precisely,we consider a situation in which payoffs upon peace depend on an uncertain state of the world about which players obtain very informative but noisy signals.Because players do not have the same assessment of the state of the world,this creates strategic uncertainty in equilibrium.At a state around which behavior switches,there will be a high probability that one player will choose peace while the other one attacks.This causes the players to second guess each other’s move,and significantly affects the sustainability of peace.These effects remain even as the players’information becomes arbitrarily precise and we approach the complete information case. Throughout the paper we compare and contrast the conditions under which cooperation is sustainable in environments with and without strategic uncertainty.To understand the difference that strategic risk makes,it is important to distinguish between the two motives for conflict that exist in this game.First,one may be tempted to attack an otherwise peaceful opponent–this is the predatory motive for conflict.Second, one may attack to avoid suffering a surprise strike from an opponent who is expected to be aggressive–this is the preemptive motive for conflict.Under complete information,it is easy for players to coordinate and only predatory motives matter.Under strategic uncertainty however,the sustainability of peace depends significantly on both predatory and preemptive incentives.Because weapon stocks can affect preemptive and predatory incentives differently, many comparative statics that were unambiguous under complete information become much more nuanced under strategic risk.Ourfirst set of results considers symmetric increases in weapon stocks.Under complete information,increased weapons stocks facilitate peace by diminishing payoffs upon conflict. Under strategic risk however,the symmetric accumulation of weapons may very well be destabilizing.Indeed,while weapons diminish predatory incentives,they may increase pre-emptive incentives if being the victim of a surprise attack is particularly weakening.It follows exit framework encompasses the insights we obtain from a repeated prisoners’dilemma.See Chassang and Takahashi(2009)for a full-fledged analysis of repeated games under related incomplete information perturbations.3See for instance Carlsson and van Damme(1993)and Morris and Shin(1998)for seminal work on global games,and Morris and Shin(2003)for a review.3that under general conditions the impact of weapons on peace will be non-monotonic.In particular,very large stocks of weapons(e.g.nuclear stocks sufficiently large to guarantee mutually assured destruction)will foster peace,whereas intermediate stocks of weapons(e.g.a few nuclear warheads that could be destroyed by a surprise strike)may be destabilizing.Our second set of results explores how inequality in military strength affects stability.It is easy to show that unequal military power is always destabilizing under complete informa-tion.This is because inequality increases the predatory temptation of the stronger player. However,inequality reduces the preemptive motive for conflict for two reasons.First,the stronger player knows she has little to fear from the weaker one and hence she has smaller preemptive needs.Second,when the strong player is overwhelmingly dominant,the weaker player can only gain very little by launching a preemptive attack.As a consequence,under strategic risk,peace might be possible between unequal contenders in circumstances under which equally armed opponents wouldfight.This result,however,should not be interpreted as making a case for complete monopoly of violence.Indeed,while inequality can help,peace is only sustainable if the weaker player keeps enough weapons to limit the stronger player’s predatory incentives.This suggests that restrained superiority may sustain the greatest level of peace.Finally,we examine the impact of peace-enforcing interventions on peace and conflict. Wefirst highlight that under complete information,unless intervention is immediate and war is prevented altogether,intervention will always have a destabilizing impact.Indeed,as in the familiar case of grim trigger strategies,it is precisely the prospect of a long and painful conflict that deters players from attacking in thefirst place.This conclusion,however,is not robust to strategic risk.By alleviating the potential costs of being the victim of a surprise attack,intervention reduces preemptive incentives.In that setting we show that the promise of intervention may promote peace even if it can only happen with delay.This paper focuses entirely on the impact of strategic risk on the mechanics of deterrence and peace.As a result,the paper abstracts from a number of other realistic dimensions of conflict already emphasized in the literature.These include several frictions that induce bar-4gaining failures,such as imperfect information(see Fearon(1995)or Powell(1999)),leader bias(see Jackson and Morelli(2007)),and commitment problems(as in Powell(2004)or Yared(2009)).Also,we do not consider the question of endogenous investment in weapons and the guns vs butter trade-off(see for instance Grossman(1991),Skaperdas(1992),Es-teban and Ray(2008),as well as Jackson and Morelli(2009)who examine a model based on this trade-offthat exhibits deterrence).Rather,our purpose here is to revisit a more primitive question:how does the accumulation of weapons affect the stability of peace?While our contribution here is mostly applied,this paper also belongs to the recent theoretical literature on dynamic global games.4It is closely related to the work of Steiner (2008),Chassang(2009),Giannitsarou and Toxvared(2009),or Ordo˜n ez(2009),all of which use a simple dynamic programming approach to simplify the analysis of large global games. In these papers,as well as in ours,payoffshocks are independent across periods and the focus is on how incomplete information affects the provision of incentives,rather than on how players may learn the underlying state of the world.A complementary literature focuses on such learning by considering dynamic global games in which the state is constant or follows a random walk.See for instance Chamley(1999),Angeletos,Hellwig and Pavan(2007), Dasgupta(2007)or Dasgupta,Steiner and Stewart(2008).Because the exit game we consider can be thought of as a reduced form for trigger strategies in a repeated game,the basic insights of the paper can be applied in other en-vironments usually modeled using repeated games.Whenever predatory and preemptive incentives move in different directions,taking strategic risk seriously will significantly affect comparative statics.One possible application is the model of price wars during booms of Rotemberg and Saloner(1986)which shows that collusion is hardest to sustain during times 4It is also useful to relate this paper to some of our other applied work on conflict.In a small extension of the current paper(Chassang and Padro i Miquel(2009a)),we use the framework developed here to discuss the relative merit of defensive weapons and defensive alliances as means to sustain peace.In an other recent paper(Chassang and Padro i Miquel(2009b))we use a complete information model to discuss the impact of wealth on conflict in a context where wealth is expropriable.We highlight that it is temporary changes in wealth,rather than the level of wealth,that determine conflict.We note that in contrast to the current paper,considerations of strategic risk do not change the intuitions obtained in the complete information setting.5of temporary high demand since this is when predatory incentives are maximized.To the extent that preemptive incentives might be highest when demand is low(failing to react might put afirm out of business),introducing strategic risk may alter comparative statics. Similarly,the relational contracting literature(see for instance,Shapiro and Stiglitz(1985), Bull(1987),Baker,Gibbons and Murphy(1994,2002),or Levin(2003))often makes the point that reducing the players’outside option facilitates cooperation.This need not hold anymore in a model with strategic risk if reducing the players’outside option increases their incentives to preempt.The paper is organized as follows.Section2describes the framework and provides neces-sary and sufficient conditions for the sustainability of peace under complete and incomplete information.Section3contrasts the mechanics of deterrence with and without strategic risk. Section4studies how inequality in military strength affects conflict.Section5explores the impact of intervention on peace.Section6concludes.Proofs are contained in Appendix A.2Framework2.1A Simple Class of Cooperation GamesWe consider two groups i∈{1,2}that play an infinite horizon trust game,with discrete time t∈N,and share a common discount factorδ.Each period t,players simultaneously decide whether to be peaceful(P)or attack(A).If both players are peaceful at time t,they obtain aflow payoffπand the game moves on to period t+1.When either of the players attacks,the game enters a conflict mode.Players receive an exogenously specified stream of payoffs and strategic interaction per-se ends.When player i attacks while−i is peaceful, she is afirst mover and gets a stream of payoffs(f i,n)n≥0,where n denotes the number of periods elapsed since conflict began.5If the opposite happens,player i is a second mover and gets a stream of payoffs(s i,n)n≥0.If both players attack at the same time,simultaneous 5i.e.if conflict started at time t,theflow payoffobtained by afirst mover i at time t+n is f i,n.6war begins and player i gets a stream of payoffs(w i,n)n≥0.We define F i,S i and W i the present discounted values of starting conflict as afirst,second or simultaneous mover.More specifically,we define,F i=+∞n=0δn f i,n;S i=+∞n=0δn s i,n;W i=+∞n=0δn w i,n.6Throughout the paper F i,S i and W i will depend on the respective stocks of weapons k i and k−i of each player.More specifically,there are functions F,S and W such that,F i=F(k i,k−i),S i=S(k i,k−i),W i=W(k i,k−i).Whenever k i=k−i=k,we use the notation F i=F(k),S i=S(k)and W i=W(k).We maintain the following assumption.Assumption1Payoffs F i,S i and W i are increasing in k i and decreasing in k−i.Further-more,F(k),S(k)and W(k)are all decreasing in k.This is a fairly natural assumption:conditional on conflict,player i’s payoffis increasing in her own stock of weapons and decreasing in her opponent’s stock of weapons.Moreover, a symmetric increase in the amount of weapons makes conflict more painful on all sides. Throughout the paper,we discuss weapon stocks k i and k−i affect the sustainability of peace under different informational environments.In any period t,given continuation values(V i)i∈{0,1}upon joint cooperation,players can 6Note that trigger strategies in a repeated game are naturally mapped into this framework.Consider for instance,in the Prisoners’Dilemma,with stage game payoffs given byP APπ−cA b0whereπ<b and b−c<2πso that peace is efficient.Trigger strategies correspond to payoffs upon conflict f i,0=b,s i,0=−c,w i,0=0and f i,n=s i,n=w i,n=0for n>0.7be thought of as facing the one-shot game,P APπ+δV i S iA F i W iwhere payoffs are given for row player i.7This representation of payoffs allows us to identify two distinct motives for conflict.The payoffdifference F i−π−δV i corresponds to player i’s predatory incentives,that is,how much player i would gain from attacking a consistently peaceful opponent.When players expect permanent peace upon continuation,predatoryπ.The payoffdifference W i−S i corresponds to the preemptive incentives take the form F i−11−δincentives of player i,that is,how much player i would gain from attacking an opponent that is expected to attack.We make the following assumption.Assumption2(early mover advantage)For all i∈{1,2},F i>W i>S i. Assumption2simply states that if conflict occurs,there is an advantage to attacking early. This assumption is natural in many instances of conflict,including military conflict,conflict betweenfirms,or even conflict between individuals,as thefirst mover benefits from additional time to prepare her moves.Throughout the paper,we contrast a situation in which theflow benefits of peaceπare common knowledge,and a situation in which players make noisy but precise private assessments of the value ofπ.In thefirst case,common knowledge of payoffs allows players to coordinate their actions effectively and only predatory incentives matter for the sustainability of peace.Under incomplete information however,coordination becomes difficult as players attempt to second guess one another’s value for peace.In that case the sustainability of peace depends significantly on both predatory and preemptive incentives.Note that while we emphasize the players’uncertainty over the common returns to peace 7We look at a situation where the benefits of cooperationπare symmetric for the purpose of simplicity. Extending the model to a setting with asymmetric benefits presents no conceptual difficulty and simply adds to the notational burden.8π,our results would be identical if we consider uncertainty over the returns F from a surprise attack.8Indeed,it is uncertainty over predatory incentives F−π−δV i as a whole that drives our results.Note in addition that unfavorable economic shocks are in fact a major driver of conflict(see Miguel et al(2004)or Ciccone(2008)).2.2The Complete Information BenchmarkIn the benchmark complete information setting,payoffπisfixed and common knowledge among players.We denote byΓCI the corresponding dynamic game.Proposition1(cooperation under complete information)Peace is(permanently)sus-tainable in an equilibrium ofΓCI if and only if∀i∈{1,2},F i−11−δπ≤0.(1)This means that under complete information,the sustainability of peace depends only on the magnitude of predatory incentives.Preemptive incentives play no role as neither S i nor W i enter condition(1).Note that this condition is analogous to the condition for cooperation in a Prisoners’Dilemma under grim trigger strategies.We denote byπCI the smallest value ofπsuch that inequality(1)holds.Let us turn to the case of strategic risk.2.3Strategic RiskWe model strategic risk in equilibrium by allowing players to have different perceptions of their environment.Although strategies are common knowledge in equilibrium,the fact that perceptions are private implies that there is no common knowledge of what actions will be taken.This leads players to try to second guess each other’s next move in order to avoid suffering a surprise attack.This second guessing is closely related to the idea of“reciprocal 8See Chassang(2009)for a general framework in which perturbations can affect all entries of the payoffmatrix.9fear of surprise attacks”developed by Schelling(1960).We are ultimately interested in determining when such thought processes lead to an unraveling of peace.9 We consider an environment in which the returns to peace are not common knowledge. Specifically,we follow the framework of Chassang(2009)and consider the slightly perturbed exit game withflow payoffsP AP˜πt S iA F i W iwhere˜πt is an i.i.d.random variable withfinite variance,distribution g and support (−∞,+∞).The payoffof cooperation˜πt is not directly observable by the players when they make their decision at time t.Instead,players observe signals of the form x i,t=˜πt+σ i,t where{ i,t}i∈{1,2},t∈N is an i.i.d.sequence of centered errors with support[−1,1],andσ>0. For simplicity we assume that˜πt is observable in period t+1via theflow payoffs.Let us denote this game byΓσ,g.To perform a robustness check on the complete information environment we are interested in the sustainability of peace inΓσ,g asfirst,σgoes to0,and second,g approaches a point mass atπ.10This corresponds to an environment where players have approximately complete information about the state of the world,but remain uncertain about whether they are more or less optimistic than the other player.Analysis is facilitated by the fact that given a distribution g,asσbecomes small,gameΓσ,g admits a most peaceful equilibrium s Hσ,gwhichsustains the highest equilibrium values V Hσ,g .Equilibrium s Hσ,galso takes a simple thresholdform,i.e.,there exists(x Hi,σ,g)i∈{1,2}∈R2such that player i plays peace whenever she gets asignal x i,t≥x Hi,σ,gand attacks otherwise.119For a related model of reciprocal fears see Baliga and Sjostr¨o m(2004).10Note that the order of limits we take is important.By takingσto0first,we insure that the players always care about their private information,so that there is indeed second guessing and strategic risk.When we take the other order of limits,the players have such strong priors that they regard their private signals as completely noisy and we are essentially back in the complete information setting.11See the appendix for more formal statements and proofs.It is important to note that we do not restrict10Before characterizing this most peaceful equilibrium in the limit case where players have very precise information,it is useful to delineate why a small amount of incomplete infor-mation can radically affect equilibrium behavior.For this purpose let us focus on the case where payoffs and signalling structures are symmetric.In that setting,the most peaceful equilibrium is symmetric with both players using the same threshold x H.Withσsmall,σ,ghas little uncertainty about her a player that gets a signal well below or well above x Hσ,gopponent’s behavior.The likelihood of surprise attacks is small.However,when a player,then there is roughly probability a half that her opponent gets as a signal the threshold x Hσ,ggot a higher signal and probability a half that her opponent got a lower signal.This means that an equilibrium threshold must be such that at that state of the world,a player is willing to be peaceful even though there is probability roughly a half that her opponent will launch a surprise attack.Note that in aggregate,the overall probability of a surprise attack maybe vanishing.What matters is that conditional on being at an equilibrium threshold,there is a high likelihood of an attack.This is why a small amount of incomplete information can significantly affect the way players interact even though,in aggregate,surprise attacks are quite rare.We now characterize explicitly when peace can be sustained under strategic risk.For this purpose we introduce some notation.Given any pair V=(V i,V−i)of continuation values, we consider the following2×2game G(V)P APπ+δV i S iA F i W i,where payoffs are given for row player i.Following Harsanyi and Selten(1988),we say that attention to threshold-form strategies.Rather,we prove that gameΓσ,g admits a most peaceful equilibrium, and that this equilibrium is necessarily in threshold-form strategies.11(P eace,P eace)is risk-dominant in game G(V)if and only ifi∈{1,2}(π+δV i−F i)+>i∈{1,2}(W i−S i).Inversely,we say that(Attack,Attack)is risk-dominant if the opposite strict inequality holds. We also denote byV≡11−δπthe value of permanent peace.We can now state the main result of this section,which we use throughout the paper.Recall that V Hσ,gdenotes the highest equilibrium pair of values in gameΓσ,g.It is supported by the most cooperative equilibrium.Proposition2(cooperation under strategic risk)For any sequence{g n}n∈N such that for all n∈N,g n has support(−∞,+∞)and{g n}n∈N converges in mean to the unit mass atπ,the following hold:(i)Whenever(P eace,P eace)is risk-dominant in game G(V,V),then permanentpeace is sustainable under strategic risk,in the sense thatlim n→∞limσ→0V Hσ,g n=V,V.(ii)Inversely,whenever(Attack,Attack)is risk-dominant in game G(V,V),then peace is unsustainable under strategic risk,in the sense thatlim n→∞limσ→0V Hσ,g n=(W i,W−i).Proposition2provides a convenient criterion to check whether peace is sustainable under strategic risk.Point(i)shows that when(P eace,P eace)is risk-dominant in G(V,V),then the highest sustainable equilibrium value inΓσ,g converges to the value of permanent peace V,which implies that the most cooperative equilibrium ofΓσ,g sustains approximately per-12manent peace.Inversely,point(ii)shows that when(Attack,Attack)is risk dominant,then the values associated with the most cooperative equilibrium ofΓσ,g converge to the value of immediate conflict.This implies that permanent conflict is the only equilibrium sustainable under strategic risk.Altogether,points(i)and(ii)imply that peace is robust to strategicrisk if and only ifi∈{1,2}11−δπ−F i+>i∈{1,2}(W i−S i)(2)where(z)+≡max{0,z}.Let us denote byπSR the smallest value ofπsuch that(2)holds.12 Condition(2)shows that just as under complete information,it is necessary that both players’predatory incentives(F i−1π)be negative to sustain peace.13In addition,condition (2)emphasizes the role of preemptive incentives(W i−S i).The larger preemptive incentives are,the harder it is to sustain peace.When payoffs are symmetric,peace is sustainable under strategic risk if and only if F−11−δπ+W−S<0,i.e.peace is sustainable if and only if the sum of predatory and preemptive incentives is negative.As Sections3,4and5show,there will often be a conflict between minimizing predatory incentives and minimizing preemptive incentives.As a consequence,taking strategic risk seriously can refine in important ways our understanding of cooperation and conflict.From a modeling perspective,we consider the limit where the distribution g of returns from peace˜πt becomes concentrated around a given valueπboth for the purpose of tractabil-ity and because it allows us to focus exclusively on the role of preemptive incentives in determining the players’ability to cooperate.A drawback of taking this limit is that in our model,conflict either begins in thefirst period(if(P eace,P eace)is not risk-dominant in G(V,V)),or the likelihood of conflict infinite time is zero(if(P eace,P eace)is risk-dominant in G(V,V)).It is not difficult to resolve this problem since our analysis extends 12Note that the equilibrium in which players always attack is always robust to strategic risk.In fact,“attacking always”is an equilibrium ofΓσ,g for allσand all g.As Chassang(2009)notes,in games with an infinite horizon,the global games perturbation cannot be used as a trick to select a unique equilibrium. Rather,the global games perturbation serves as a model of strategic risk in equilibrium that introduces preemption as a motive for conflict.13Indeed,since W i−S i>0,Condition(2)holds only if11−δπ−F i>0for i∈{1,2}.13easily to circumstances where information is precise but the distribution g is not degenerate (see Chassang(2009)).In that case,the most peaceful equilibrium will still be in threshold strategies,but there will be some probability of conflict in each period depending on whether the realized return to peace˜πt is above the threshold or not.Most importantly,the equilib-rium threshold will still be determined by risk-dominance concerns and the broad qualitative points we make in the paper would be unchanged.However,because payoffs upon conflict would now enter continuation values upon peace and change the potential surplus available in the game,the analysis would become richer and obscure the role played by preemptive incentives.3Deterrence with Symmetric Weapon Stocks3.1General ResultsThis section investigates how a symmetric increase in weapon stocks affects the sustainability of peace by studying the comparative statics of thresholdsπCI andπSR.These thresholds correspond respectively to the minimumflow returns to peaceπnecessary for peace to be sustainable under complete information and under strategic risk.This implies that the lower πCI andπSR are,the easier it is to sustain peace.We say that weapons are deterrent if and only if the symmetric accumulation of weapons reduces the minimum value ofπrequired to sustain peace.The following proposition describes how the deterrent effect of weapons may differ across strategic settings.Recall that payoffs upon conflict F i,S i and W i depend on the players’respective weapon stocks,k i and k−i.In addition,when weapon stocks are symmetric,i.e. k i=k−i=k,then all payoffs upon conflict are decreasing in k.Proposition3(deterrence under complete and incomplete information)Consider a situation in which k i=k−i=k.We have that(i)πCI is always strictly decreasing in k.14。
Submanifolds of generalized complex manifolds

arXiv:math/0309013v1 [math.DG] 1 Sep 2003
OREN BEN-BASSAT AND MITYA BOYARCHENKO Abstract. The main goal of our paper is the study of several classes of submanifolds of generalized complex manifolds. Along with the generalized complex submanifolds defined by Gualtieri and Hitchin in [4], [8] (we call these “generalized Lagrangian submanifolds” in our paper), we introduce and study three other classes of submanifolds. For generalized complex manifolds that arise from complex (resp., symplectic) manifolds, all three classes specialize to complex (resp., symplectic) submanifolds. In general, however, all three classes are distinct. We discuss some interesting features of our theory of submanifolds, and illustrate them with a few nontrivial examples. We then support our “symplectic/Lagrangian viewpoint” on the submanifolds introduced in [4], [8] by defining the “generalized complex category”, modelled on the constructions of Guillemin-Sternberg [5] and Weinstein [14]. We argue that our approach may be useful for the quantization of generalized complex manifolds.
The homoclinic and heteroclinic C-algebras of a generalized one-dimensional solenoid

KLAUS THOMSEN
a 2008
1. Introduction The homoclinic and heteroclinic structure in dynamical systems was first used to produce C ∗ -algebras in the way breaking work of Cuntz and Krieger in [Kr1], [Kr2] and [CuK]. This work has been generalized in many directions where the relation to dynamical systems is either absent or appears very implicit, but Ian Putnam described in [Pu] a natural way to extend the constructions of Cuntz and Krieger to higher dimensions such that the point of departure is the heteroclinic structure in a Smale space, just as the work of Cuntz and Krieger departed from the heteroclinic structure in a shift of finite type, which is a zero-dimensional Smale space. Putnam builds his approach on the work of D. Ruelle, [Ru1], [Ru2], who introduced the notion of a Smale space in [Ru1] and constructed the so-called asymptotic algebra from the homoclinic equivalence relation in [Ru2]. The work of Putnam and Ruelle was further generalized by the author in [Th1] where it was shown that Ruelle’s approach can be adopted as soon as there is enough expansiveness in the underlying dynamical system; the local product structure in a Smale space is not crucial for the construction. Furthermore, in [Th1] the alternative approach was used to obtain inductive limit decompositions for the algebras of Putnam arising from particular classes of Smale spaces, e.g. expansive group automorphisms and one-dimensional generalized solenoids in the sense of R.F. Williams, [Wi2], and I. Yi, [Y1]. For expansive group automorphisms it was shown that the C ∗ -algebras are all AT-algebras of real rank zero, and hence are classified by their K-theory groups, thanks to the work of G. Elliott, [Ell1]. For one-dimensional generalized solenoids the exact nature of the inductive limit decomposition was not determined and the homoclinic algebra was not examined. In particular, it was not decided if the C ∗ -algebras are classified by K-theory. The main purpose of the present paper is tie up this loose end by showing that they are, although they turn out to be more general AH-algebras and exhibit more complicated K-theory than the algebras arising from expansive group automorphisms, at least in the sense that torsion appears. Specifically, it is shown that the heteroclinic algebra of both a one-solenoid and its inverse, as well as the homoclinic algebra are all AH-algebras of real rank zero with no dimension growth. They are therefore classified by K-theory thanks to the work of Elliott and Gong, [EG]. This conclusion is obtained for the heteroclinic algebra by combining a thorough study of the inductive limit decomposition obtained in [Th1] with results on the classification of simple C ∗ -algebras, in particular results by H. Lin on algebras of tracial rank zero, cf. e.g. [Lin4].
西方文论原典导读尔雅满分答案

~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~ ------------------------------------------------------------------------------------------------Lecture 11___B__created the world famous Athens school.A、SocratesB、PlatoC、Aristotle2Socrates’ theory is mainly organized by whom?AA、PlatoB、AristotleC、Thales3Ion as a poet, what did he sing when he won the laurel?BA、tragedyB、homericC、comedy4Socrates said that when the inspiration came, the poets will sink into __C__.A、melancholyB、angerC、ecstasy5In Ion Socrates stressed that the inspiration of the poets comes from the __A__.A、godsB、heartC、experience6__A___ said “I am the midwive of philosophy”.A、SocratesB、PlatoC、Aristotle7Who was the first to pick out eidos?BA、SocratesB、PlatoC、Aristotle8What is subject matter of Ion?CA、WealthB、Knowledge9Three sages of Ancient Greece are Plato,__B___and Aristotle.A、ProtagorasB、SocratesC、Homer10Plato use the __A___ to explain the relationship between art and idea.A、bedB、tableC、bookshelfLecture 21Aristotle’s poetics was valued in the history of western literary criticism from __C___century in France.A、13thB、14thC、15th2Until Aristotle, the concept of ___B__ has been adressed explicitly.A、structureB、system3In Aristotle’s view, the core of art is ___A__.A、imitationB、emotionC、truth4In Aristotle’s words, who is more to the truth than historian?BA、painterB、poetC、playwright5__C___ is called a Encyclopedia of character in Greek.A、SocratesB、PlatoC、Aristotle6Compared with Plato, the biggest character of Aristotle is___A__.A、comprehensivenessB、scientifictyC、far-reaching7Aristotle thinks types differ from one another in three ways: the means, objects and __C___.A、structureB、frameC、manner8Aristotle’s Poetics maining discussed about ___A__ and epic.A、tragedyB、comedyC、prose9In the poetics, __A___ is the goal of the tragedy.A、plotB、ethosC、pathos10Aristotle set up his own schools called ___A__.A、lyceumB、athensC、freedomLecture 31In__A___,Samuel started compiling a dictionary of the Engish language.A、1747B、1755C、1765.02What’s the attitude on Shakespeare by Samuel Johnson?CA、criticalB、praisableC、A and B3How to treat the relationship between Johson and classical philosophy.BA、full acceptedB、eclecticC、rejected4Which work of Sumel that even plexander praised it.AA、londonB、the iderC、the rambler5Johson considered the shortcoming of Shakespeare is losing of___B__.A、pleasureB、teachingC、conflict6获取完整版答案,请打开微信扫一扫下方二维码,关注微信公众号:帮帮ING如果无法扫码关注,请按照下方提示操作:In__B___ Jhnson finally published essays of Shakespeare’s plays.A、1755B、1765C、1775.07Samuel Johnson was known in review of _B____.A、HomerB、ShakespeareC、Plato8Characteristics of Johson’s theory on Shakespeare is__A___.A、not persistentB、straightforwardC、obscare9__A___ is a friend and biographyer for Samuel.A、James BoswellB、David GarrickC、Edward Cave10Which work is written by Samuel?BA、Words and objectsB、The vanity of human wishesC、PanopticonLecture 41How does Wordsworth think about the relationship between Poetic language and Prose language?AA、no essential differenceB、unrelatedC、mutually exclusive2Wordsworth compares poetry to___B__ when he talked about the function of poetry.A、prismB、bedC、weapon3Wordsworth gained his reputation among chinese scholars by “Poetry is the nature explession of ___A__”.A、strong emotionsB、external stimuliC、language structure4In the Mirror and the lamp, wordsworth is concluded as a representative of __A___ theories.A、expressiveB、romanticC、emotional5Which book is the representative work of english romantic movement?AA、Lyrical Ballads(1798)B、Lyrical Ballads(1800)C、Beside the tintern abbey6There are two themes of poetry in Wordsworth’s view___C__ and the feeling of the common people.A、villageB、cityC、nature7In what year that wordsworth published his Preface to Lyrical Ballads.BA、1798B、1800C、1802.08Wordsworth’s philosophical views almost come from ___B__.A、theory of evolutionB、the apocalypse of natureC、coleridge9Wordsworth, Coleridgo and southey are called __A___.A、lake poetsB、literature threeC、absurdist poets10Which work was published by Wordsworth’s widow after his death.BA、Lyrical BalladsB、The preludeC、Solitary Reaper11From the aspect of words, it emphasized words__C__.A、close to lifeB、unnaturalC、classicalLecture 51Which work is not written by Taine.CA、The philosophy of artB、The ideal in artC、Biographia literaria2Taine’s theory enlightened people to pay close attention to ___A__ factors of art in his time.A、externalB、internalC、structural3The 3 aspects by Taine are the race, the __C___ and the epoch.A、populationB、polityC、surroundings4Taine believed that literary studies should be based on the _B___ vision.A、scientificB、historicalC、realistic5Which is not the origin of Taine’s theory in a literary perspective?CA、Sainte-beuveB、Madame de stealC、Rousseau6Taine is called as “critics of __A___”.A、NapoleonB、CharlieC、Hitler7In Taine’s first aspect, which country makes philosophers.CA、FranceB、EnglandC、Germany8Which method in 19th century made a great influence on Taine’s theory?AA、scientific methodB、historical methodC、artistic method9In the race,circumstance,and epoch,which is the acquired momentum.CA、raceB、circumstanceC、epoch10In Taine’s view, race is closely related to __A___.A、hereditaryB、individualityC、diversity11Tain e’s theory of surroundings,shows that he thinks the world__B___.A、is diversifiedB、has a whole relationshipC、has different elements。
On the interplay between measurable and topological dynamics

a rX iv:mat h /48328v1[mat h.DS]24Aug24ON THE INTERPLAY BETWEEN MEASURABLE AND TOPOLOGICAL DYNAMICS E.GLASNER AND B.WEISS Contents Introduction 2Part 1.Analogies 31.Poincar´e recurrence vs.Birkhoff’s recurrence 31.1.Poincar´e recurrence theorem and topological recurrence 31.2.The existence of Borel cross-sections 41.3.Recurrence sequences and Poincar´e sequences 52.The equivalence of weak mixing and continuous spectrum 73.Disjointness:measure vs.topological d mixing:measure vs.topological 125.Distal systems:topological vs.measure 196.Furstenberg-Zimmer structure theorem vs.its topological PI version 217.Entropy:measure and topological 227.1.The classical variational principle 227.2.Entropy pairs and UPE systems 227.3.A measure attaining the topological entropy of an open cover 237.4.The variational principle for open covers 287.5.Further results connecting topological and measure entropy 307.6.Topological determinism and zero entropy 31Part 2.Meeting grounds 338.Unique ergodicity 339.The relative Jewett-Krieger theorem3410.Models for other commutative diagrams3911.The Furstenberg-Weiss almost 1-1extension theorem4012.Cantor minimal representations4013.Other related theorems41References442 E.GLASNER AND B.WEISSIntroductionRecurrent-wandering,conservative-dissipative,contracting-expanding,deter-ministic-chaotic,isometric-mixing,periodic-turbulent,distal-proximal,the list can go on and on.These(pairs of)words—all of which can be found in the dictio-nary—convey dynamical images and were therefore adopted by mathematicians to denote one or another mathematical aspect of a dynamical system.The two sister branches of the theory of dynamical systems called ergodic theory(or measurable dynamics)and topological dynamics use these words to describe different but parallel notions in their respective theories and the surprising fact is that many of the corresponding results are rather similar.In the following article we have tried to demonstrate both the parallelism and the discord between ergodic theory and topo-logical dynamics.We hope that the subjects we chose to deal with will successfully demonstrate this duality.The table of contents gives a detailed listing of the topics covered.In thefirst part we have detailed the strong analogies between ergodic theory and topological dynamics as shown in the treatment of recurrence phenomena,equicontinuity and weak mixing,distality and entropy.In the case of distality the topological version camefirst and the theory of measurable distality was strongly influenced by the topo-logical results.For entropy theory the influence clearly was in the opposite direction. The prototypical result of the second part is the statement that any abstract mea-sure probability preserving system can be represented as a continuous transformation of a compact space,and thus in some sense ergodic theory embeds into topological dynamics.We have not attempted in any way to be either systematic or comprehensive. Rather our choice of subjects was motivated by taste,interest and knowledge and to great extent is random.We did try to make the survey accessible to non-specialists, and for this reason we deal throughout with the simplest case of actions of Z.Most of the discussion carries over to noninvertible mappings and to R actions.Indeed much of what we describe can be carried over to general amenable groups.Similarly, we have for the most part given rather complete definitions.Nonetheless,we did take advantage of the fact that this article is part of a handbook and for some of the definitions,basic notions and well known results we refer the reader to the earlier introductory chapters of volume I.Finally,we should acknowledge the fact that we made use of parts of our previous expositions[86]and[35].We made the writing of this survey more pleasurable for us by the introduction of a few original results.In particular the following results are entirely or partially new.Theorem1.2(the equivalence of the existence of a Borel cross-section with the coincidence of recurrence and periodicity),most of the material in Section4 (on topological mild-mixing),all of subsection7.4(the converse side of the local variational principle)and subsection7.6(on topological determinism).MEASURABLE AND TOPOLOGICAL DYNAMICS3 Part1.Analogies1.Poincar´e recurrence vs.Birkhoff’s recurrence1.1.Poincar´e recurrence theorem and topological recurrence.The simplest dynamical systems are the periodic ones.In the absence of periodicity the crudest approximation to this is approximate periodicity where instead of some iterate T n x returning exactly to x it returns to a neighborhood of x.Thefirst theorem in abstract measure dynamics is Poincar´e’s recurrence theorem which asserts that for afinite measure preserving system(X,B,µ,T)and any measurable set A,µ-a.e.point of A returns to A(see[46,Theorem4.3.1]).The proof of this basic fact is rather simple and depends on identifying the set of points W⊂A that never return to A.These are called the wandering points and their measurability follows from the formulaW=A∩ ∞ k=1T−k(X\A) .Now for n≥0,the sets T−n W are pairwise disjoint since x∈T−n W means that the forward orbit of x visits A for the last time at moment n.Sinceµ(T−n W)=µ(W) it follows thatµ(W)=0which is the assertion of Poincar´e’s theorem.Noting that A∩T−n W describes the points of A which visit A for the last time at moment n, and thatµ(∪∞n=0T−n W)=0we have established the following stronger formulation of Poincar´e’s theorem.1.1.Theorem.For afinite measure preserving system(X,B,µ,T)and any measur-able set A,µ-a.e.point of A returns to A infinitely often.Note that only sets of the form T−n B appeared in the above discussion so that the invertibility of T is not needed for this result.In the situation of classical dynam-ics,which was Poincar´e’s main interest,X is also equipped with a separable metric topology.In such a situation we can apply the theorem to a refining sequence of partitions P m,where each P m is a countable partition into sets of diameter at most1m ofitself,and since the intersection of a sequence of sets of full measure has full measure, we deduce the corollary thatµ-a.e.point of X is recurrent.This is the measure theoretical path to the recurrence phenomenon which depends on the presence of afinite invariant measure.The necessity of such measure is clear from considering translation by one on the integers.The system is dissipative,in the sense that no recurrence takes place even though there is an infinite invariant measure.There is also a topological path to recurrence which was developed in an abstract setting by G.D.Birkhoff.Here the above example is eliminated by requiring that the topological space X,on which our continuous transformation T acts,be compact.It is possible to show that in this setting afinite T-invariant measure always exists,and so we can retrieve the measure theoretical picture,but a purely topological discussion will give us better insight.4 E.GLASNER AND B.WEISSA key notion here is that of minimality.A nonempty closed,T-invariant set E⊂X, is said to be minimal if F⊂E,closed and T-invariant implies F=∅or F=E.If X itself is a minimal set we say that the system(X,T)is a minimal system. Fix now a point x0∈X and consider∞ n=1ω(x0)=MEASURABLE AND TOPOLOGICAL DYNAMICS5 that the converse is also valid—namely if there are no conservative quasi-invariant measures then there is a Borel cross-section.Note that the periodic points of(X,T)form a Borel subset for which a cross-section always exists,so that we can conclude from the above discussion the following statement in which no explicit mention is made of measures.1.2.Theorem.For a system(X,T),with X a completely metrizable separable space, there exists a Borel cross-section if and only if the only recurrent points are the peri-odic ones.1.3.Remark.Already in[42]as well as in[21]onefinds many equivalent conditions for the existence of a Borel section for a system(X,T).However one doesn’tfind there explicit mention of conditions in terms of recurrence.Silvestrov and Tomiyama [76]established the theorem in this formulation for X compact(using C∗-algebra methods).We thank zar for drawing our attention to their paper.1.3.Recurrence sequences and Poincar´e sequences.We will conclude this sec-tion with a discussion of recurrence sequences and Poincar´e sequences.First for some definitions.Let us say that D is a recurrence set if for any dynamical system(Y,T) with compatible metricρand anyǫ>0there is a point y0and a d∈D withρ(T d y0,y0)<ǫ.Since any system contains minimal sets it suffices to restrict attention here to minimal systems.For minimal systems the set of such y’s for afixedǫis a dense open set. To see this fact,let U be an open set.By the minimality there is some N such that for any y∈Y,and some0≤n≤N,we have T n y∈ing the uniform continuity of T n,wefind now aδ>0such that ifρ(u,v)<δthen for all0≤n≤Nρ(T n u,T n v)<ǫ.Now let z0be a point in Y and d0∈D such that(1)p(T d0z0,z0)<δ.For some0≤n0≤N we have T n0z0=y0∈U and from(1)we getρ(T d0y0,y0)<ǫ. Thus points thatǫreturn form an open dense set.Intersecting overǫ→0gives a dense Gδin Y of points y for whichρ(T d y,y)=0.infd∈DThus there are points which actually recur along times drawn from the given recur-rence set.A nice example of a recurrence set is the set of squares.To see this it is easier to prove a stronger property which is the analogue in ergodic theory of recurrence sets.1.4.Definition.A sequence{s j}is said to be a Poincar´e sequence if for anyfinite measure preserving system(X,B,µ,T)and any B∈B with positive measure we haveµ(T s j B∩B)>0for some s j in the sequence.6 E.GLASNER AND B.WEISSSince any minimal topological system(Y,T)hasfinite invariant measures with global support,µany Poincar´e sequence is recurrence sequence.Indeed for any presumptive constant b>0which would witness the non-recurrence of{s j}for(Y,T), there would have to be an open set B with diameter less than b and having positiveµ-measure such that T s j B∩B is empty for all{s j}.Here is a sufficient condition for a sequence to be a Poincar´e sequence:1.5.Lemma.If for everyα∈(0,2π)limn→∞1nn1U s k(1B−f0) L2−→0or1nn1µ(B∩T−s k B)= f0 2>0which clearly implies that{s k}is a Poincar´e sequence. The proof we have just given is in fact von-Neumann’s original proof for the mean ergodic theorem.He used the fact that N satisfies the assumptions of the proposition, which is Weyl’s famous theorem on the equidistribution of{nα}.Returning to the squares Weyl also showed that{n2α}is equidistributed for all irrationalα.For rationalαthe exponential sum in the lemma needn’t vanish,however the recurrence along squares for the rational part of the spectrum is easily verified directly so that we can conclude that indeed the squares are a Poincar´e sequence and hence a recurrence sequence.The converse is not always true,i.e.there are recurrence sequences that are not Poincar´e sequences.This wasfirst shown by I.Kriz[60]in a beautiful example(see also[86,Chapter5]).Finally here is a simple problem.MEASURABLE AND TOPOLOGICAL DYNAMICS7 Problem:If D is a recurrence sequence for all circle rotations is it a recurrence set?A little bit of evidence for a positive answer to that problem comes from looking at a slightly different characterization of recurrence sets.Let N denote the collection of sets of the formN(U,U)={n:T−n U∩U=∅},(U open and nonempty),where T is a minimal transformation.Denote by N∗the subsets of N that have a non-empty intersection with every element of N.Then N∗is exactly the class of recurrence sets.For minimal transformations,another description of N(U,U)is obtained by fixing some y0and denotingN(y0,U)={n:T n y0∈U}Then N(U,U)=N(y0,U)−N(y0,U).Notice that the minimality of T implies that N(y0,U)is a syndetic set(a set with bounded gaps)and so any N(U,U)is the set of differences of a syndetic set.Thus N consists essentially of all sets of the form S−S where S is a syndetic set.Given afinite set of real numbers{λ1,λ2,...,λk}andǫ>0setV(λ1,λ2,...,λk;ǫ)={n∈Z:maxj{ nλj <ǫ}},where · denotes the distance to the closest integer.The collection of such sets forms a basis of neighborhoods at zero for a topology on Z which makes it a topological group.This topology is called the Bohr topology.(The corresponding uniform structure is totally bounded and the completion of Z with respect to it is a compact topological group called the Bohr compactification of Z.)Veech proved in[78]that any set of the form S−S with S⊂Z syndetic contains a neighborhood of zero in the Bohr topology up to a set of zero density.It is not known if in that statement the zero density set can be omitted.If it could then a positive answer to the above problem would follow(see also[32]).2.The equivalence of weak mixing and continuous spectrumIn order to analyze the structure of a dynamical system X there are,a priori,two possible approaches.In thefirst approach one considers the collection of subsystems Y⊂X(i.e.closed T-invariant subsets)and tries to understand how X is built up by these subsystems.In the other approach one is interested in the collection of factors Xπ→Y of the system X.In the measure theoretical case thefirst approach leads to the ergodic decomposition and thereby to the study of the“indecomposable”or ergodic components of the system.In the topological setup there is,unfortunately,no such convenient decomposition describing the system in terms of its indecomposable parts and one has to use some less satisfactory substitutes.Natural candidates for in-decomposable components of a topological dynamical system are the“orbit closures”(i.e.the topologically transitive subsystems)or the“prolongation”cells(which often coincide with the orbit closures),see[4].The minimal subsystems are of particular importance here.Although we can not say,in any reasonable sense,that the study of the general system can be reduced to that of its minimal components,the analysis of8 E.GLASNER AND B.WEISSthe minimal systems is nevertheless an important step towards a better understanding of the general system.This reasoning leads us to the study of the collection of indecomposable systems (ergodic systems in the measure category and transitive or minimal systems in thetopological case)and their factors.The simplest and best understood indecomposable dynamical systems are the ergodic translations of a compact monothetic group(a cyclic permutation on Z p for a prime number p,the“adding machine”on ∞n=0Z2, an irrational rotation z→e2πiαz on S1={z∈C:|z|=1}etc.).It is not hard toshow that this class of ergodic actions is characterized as those dynamical systems which admit a model(X,X,µ,T)where X is a compact metric space,T:X→X a surjective isometry andµis T-ergodic.We call these systems Kronecker or isometric systems.Thus ourfirst question concerning the existence of factors should be:given an ergodic dynamical system X which are its Kronecker factors?Recall that a measure dynamical system X=(X,X,µ,T)is called weakly mixing if the product system(X×X,X⊗X,µ×µ,T×T)is ergodic.The following classical theorem is due to von Neumann.The short and elegant proof we give was suggested by Y.Katznelson.2.1.Theorem.An ergodic system X is weakly mixing iffit admits no nontrivial Kronecker factor.Proof.Suppose X is weakly mixing and admits an isometric factor.Now a factor of a weakly mixing system is also weakly mixing and the only system which is both isometric and weakly mixing is the trivial system(an easy exercise).Thus a weakly mixing system does not admit a nontrivial Kronecker factor.For the other direction,if X is non-weakly mixing then in the product space X×X there exists a T-invariant measurable subset W such that0<(µ×µ)(W)<1.For every x∈X let W(x)={x′∈X:(x,x′)∈W}and let f x=1W(x),a function in L∞(µ).It is easy to check that U T f x=f T−1x so that the mapπ:X→L2(µ)defined byπ(x)=f x,x∈X is a Borel factor map.Denotingπ(X)=Y⊂L2(µ),andν=π∗(µ),we now have a factor mapπ:X→(Y,ν).Now the function π(x) is clearly measurable and invariant and by ergodicity it is a constantµ-a.e.;say π(x) =1. The dynamical system(Y,ν)is thus a subsystem of the compact dynamical system (B,U T),where B is the unit ball of the Hilbert space L2(µ)and U T is the Koopman unitary operator induced by T on L2(µ).Now it is well known(see e.g.[35])that a compact topologically transitive subsystem which carries an invariant probability measure must be a Kronecker system and our proof is complete.Concerning the terminology we used in the proof of Theorem2.1,B.O.Koopman, a student of G.D.Birkhoffand a co-author of both Birkhoffand von Neumann introduced the crucial idea of associating with a measure dynamical system X= (X,X,µ,T)the unitary operator U T on the Hilbert space L2(µ).It is now an easy matter to see that Theorem2.1can be re-formulated as saying that the system X is weakly mixing iffthe point spectrum of the Koopman operator U T comprises the single complex number1with multiplicity1.Or,put otherwise,that the one dimensional space of constant functions is the eigenspace corresponding to the eigenvalue1(thisMEASURABLE AND TOPOLOGICAL DYNAMICS9 fact alone is equivalent to the ergodicity of the dynamical system)and that the restriction of U T to the orthogonal complement of the space of constant functions has a continuous spectrum.We now consider a topological analogue of this theorem.Recall that a topo-logical system(X,T)is topologically weakly mixing when the product system (X×X,T×T)is topologically transitive.It is equicontinuous when the family {T n:n∈Z}is an equicontinuous family of maps.Again an equivalent condition is the existence of a compatible metric with respect to which T is an isometry.And,moreover,a minimal system is equicontinuous iffit is a minimal translation on a compact monothetic group.We will need the following lemma.2.2.Lemma.Let(X,T)be a minimal system and f:X→R a T-invariant function with at least one point of continuity(for example this is the case when f is lower or upper semi-continuous or more generally when it is the pointwise limit of a sequence of continuous functions),then f is a constant.Proof.Let x0be a continuity point and x an arbitrary point in X.Since{T n x: n∈Z}is dense and as the value f(T n x)does not depend on n it follows that f(x)=f(x0).2.3.Theorem.Let(X,T)be a minimal system then(X,T)is topologically weakly mixing iffit has no non-trivial equicontinuous factor.Proof.Suppose(X,T)is minimal and topologically weakly mixing and letπ:(X,T)→(Y,T)be an equicontinuous factor.If(x,x′)is a point whose T×T orbit is dense in X×X then(y,y′)=(π(x),π(x′))has a dense orbit in Y×Y.However,if(Y,T) is equicontinuous then Y admits a compatible metric with respect to which T is an isometry and the existence of a transitive point in Y×Y implies that Y is a trivial one point space.Conversely,assuming that(X×X,T×T)is not transitive we will construct an equicontinuous factor(Z,T)of(X,T).As(X,T)is a minimal system,there exists a T-invariant probability measureµon X with full support.By assumption there exists an open T-invariant subset U of X×X,such that cls U:=M X×X.By minimality the projections of M to both X coordinates are onto.For every y∈X let M(y)={x∈X:(x,y)∈M},and let f y=1M(y)be the indicator function of the set M(y),considered as an element of L1(X,µ).Denote byπ:X→L1(X,µ)the map y→f y.We will show thatπis a continuous homomorphism,where we consider L1(X,µ)as a dynamical system with the isometric action of the group{U n T:n∈Z}and U T f(x)=f(T x).Fix y0∈X andǫ>0.There exists an open neighborhood V of the closed set M(y0)withµ(V\M(y0))<ǫ.Since M is closed the set map y→M(y),X→2X is upper semi-continuous and we can find a neighborhood W of y0such that M(y)⊂V for every y∈W.Thus for every y∈W we haveµ(M(y)\M(y0))<ǫ.In particular,µ(M(y))≤µ(M(y0))+ǫand it follows that the map y→µ(M(y))is upper semi-continuous.A simple computation shows that it is T-invariant,hence,by Lemma2.2,a constant.10 E.GLASNER AND B.WEISSWith y0,ǫand V,W as above,for every y∈W,µ(M(y)\M(y0))<ǫandµ(M(y))=µ(M(y0)),thusµ(M(y)∆M(y0))<2ǫ,i.e., f y−f y0 1<2ǫ.This proves the claim thatπis continuous.Let Z=π(X)be the image of X in L1(µ).Sinceπis continuous,Z is compact. It is easy to see that the T-invariance of M implies that for every n∈Z and y∈X, f T−n y=f y◦T n so that Z is U T-invariant andπ:(Y,T)→(Z,U T)is a homomorphism. Clearly(Z,U T)is minimal and equicontinuous(in fact isometric).Theorem2.3is due to Keynes and Robertson[57]who developed an idea of Fursten-berg,[22];and independently to K.Petersen[70]who utilized a previous work of W.A.Veech,[78].The proof we presented is an elaboration of a work of McMahon[66]due to Blanchard,Host and Maass,[13].We take this opportunity to point outa curious phenomenon which recurs again and again.Some problems in topological dynamics—like the one we just discussed—whose formulation is purely topological, can be solved using the fact that a Z dynamical system always carries an invariant probability measure,and then employing a machinery provided by ergodic theory.In several cases this approach is the only one presently known for solving the problem. In the present case however purely topological proofs exist,e.g.the Petersen-Veech proof is one such.3.Disjointness:measure vs.topologicalIn the ring of integers Z two integers m and n have no common factor if whenever k|m and k|n then k=±1.They are disjoint if m·n is the least common multiple of m and n.Of course in Z these two notions coincide.In his seminal paper of 1967[23],H.Furstenberg introduced the same notions in the context of dynamical systems,both measure-preserving transformations and homeomorphisms of compact spaces,and asked whether in these categories as well the two are equivalent.The notion of a factor in,say the measure category,is the natural one:the dynamical system Y=(Y,Y,ν,T)is a factor of the dynamical system X=(X,X,µ,T)if there exists a measurable mapπ:X→Y withπ(µ)=νthat T◦π=π◦T.A common factor of two systems X and Y is thus a third system Z which is a factor of both.A joining of the two systems X and Y is any system W which admits both as factors and is in turn spanned by them.According to Furstenberg’s definition the systems X and Y are disjoint if the product system X×Y is the only joining they admit.In the topological category,a joining of(X,T)and(Y,S)is any subsystem W⊂X×Y of the product system(X×Y,T×S)whose projections on both coordinates are full;i.e.πX(W)=X andπY(W)=Y.(X,T)and(Y,S)are disjoint if X×Y is the unique joining of these two systems.It is easy to verify that if(X,T)and(Y,S)are disjoint then at least one of them is minimal.Also,if both systems are minimal then they are disjoint iffthe product system(X×Y,T×S)is minimal.In1979,D.Rudolph,using joining techniques,provided thefirst example of a pair of ergodic measure preserving transformations with no common factor which are not disjoint[72].In this work Rudolph laid the foundation of joining theory.He introduced the class of dynamical systems having“minimal self-joinings”(MSJ),and constructed a rank one mixing dynamical system having minimal self-joinings of all orders.MEASURABLE AND TOPOLOGICAL DYNAMICS11 Given a dynamical system X=(X,X,µ,T)a probability measureλon the product of k copies of X denoted X1,X2,...,X k,invariant under the product transformation and projecting ontoµin each coordinate is a k-fold self-joining.It is called an off-diagonal if it is a“graph”measure of the formλ=gr(µ,T n1,...,T n k),i.e.λis the image ofµunder the map x→ T n1x,T n2x,...,T n k x of X into k i=1X i.The joiningλis a product of off-diagonals if there exists a partition(J1,...,J m)of {1,...,k}such that(i)For each l,the projection ofλon i∈J l X i is an off-diagonal,(ii) The systems i∈J l X i,1≤l≤m,are independent.An ergodic system X has minimal self-joinings of order k if every k-fold ergodic self-joining of X is a product of off-diagonals.In[72]Rudolph shows how any dynamical system with MSJ can be used to con-struct a counter example to Furstenberg’s question as well as a wealth of other counter examples to various questions in ergodic theory.In[52]del Junco,Rahe and Swanson were able to show that the classical example of Chac´o n[16]has MSJ,answering a question of Rudolph whether a weakly but not strongly mixing system with MSJ exists.In[38]Glasner and Weiss provide a topological counterexample,which also serves as a natural counterexample in the measure category.The example consists of two horocycleflows which have no nontrivial common factor but are nevertheless not disjoint.It is based on deep results of Ratner[71]which provide a complete description of the self joinings of a horocycleflow.More recently an even more strik-ing example was given in the topological category by E.Lindenstrauss,where two minimal dynamical systems with no nontrivial factor share a common almost1-1 extension,[63].Beginning with the pioneering works of Furstenberg and Rudolph,the notion of joinings was exploited by many authors;Furstenberg1977[24],Rudolph1979[72], Veech1982[81],Ratner1983[71],del Junco and Rudolph1987[53],Host1991 [47],King1992[58],Glasner,Host and Rudolph1992[36],Thouvenot1993[77], Ryzhikov1994[73],Kammeyer and Rudolph1995(2002)[55],del Junco,Lema´n czyk and Mentzen1995[51],and Lema´n czyk,Parreau and Thouvenot2000[62],to men-tion a few.The negative answer to Furstenberg’s question and the consequent works on joinings and disjointness show that in order to study the relationship between two dynamical systems it is necessary to know all the possible joinings of the two systems and to understand the nature of these joinings.Some of the best known disjointness relations among families of dynamical systems are the following:•id⊥ergodic,•distal⊥weakly mixing([23]),•rigid⊥mild mixing([27]),•zero entropy⊥K-systems([23]),in the measure category and•F-systems⊥minimal([23]),•minimal distal⊥weakly mixing,•minimal zero entropy⊥minimal UPE-systems([9]),12 E.GLASNER AND B.WEISSin the topological category.d mixing:measure vs.topological4.1.Definition.Let X=(X,X,µ,T)be a measure dynamical system.1.The system X is rigid if there exists a sequence n kր∞such thatlimµ(T n k A∩A)=µ(A)for every measurable subset A of X.We say that X is{n k}-rigid.2.An ergodic system is mildly mixing if it has no non-trivial rigid factor. These notions were introduced in[27].The authors show that the mild mixing property is equivalent to the following multiplier property.4.2.Theorem.An ergodic system X=(X,X,µ,T)is mildly mixing ifffor every ergodic(finite or infinite)measure preserving system(Y,Y,ν,T),the product system(X×Y,µ×ν,T×T),is ergodic.Since every Kronecker system is rigid it follows from Theorem2.1that mild mixing implies weak mixing.Clearly strong mixing implies mild mixing.It is not hard to construct rigid weakly mixing systems,so that the class of mildly mixing systems is properly contained in the class of weakly mixing systems.Finally there are mildly but not strongly mixing systems;e.g.Chac´o n’s system is an example(see Aaronson and Weiss[1]).We also have the following analytic characterization of mild mixing.4.3.Proposition.An ergodic system X is mildly mixing iffφf(n)<1,lim supn→∞for every matrix coefficientφf,where for f∈L2(X,µ), f =1,φf(n):= U T n f,f . Proof.If X→Y is a rigid factor,then there exists a sequence n i→∞such that U T n i→id strongly on L2(Y,ν).For any function f∈L20(Y,ν)with f =1, we have lim i→∞φf(n i)=1.Conversely,if lim i→∞φf(n i)=1for some n iր∞and f∈L20(X,µ), f =1,then lim i→∞U T n i f=f.Clearly f can be replaced by a bounded function and we let A be the sub-algebra of L∞(X,µ)generated by {U T n f:n∈Z}.The algebra A defines a non-trivial factor X→Y such that U T n i→id strongly on L2(Y,ν). We say that a collection F of nonempty subsets of Z is a family if it is hereditary upward and proper(i.e.A⊂B and A∈F implies B∈F,and F is neither empty nor all of2Z).With a family F of nonempty subsets of Z we associate the dual familyF∗={E:E∩F=∅,∀F∈F}.It is easily verified that F∗is indeed a family.Also,for families,F1⊂F2⇒F∗1⊃F∗2, and F∗∗=F.。
学术英语(社科)Unit2二单元原文及翻译

UNIT 2 Economist1.Every field of study has its own language and its own way of thinking. Mathematicians talk about axioms, integrals, and vector spaces. Psychologists talk about ego, id, and cognitive dissonance. Lawyers talk about venue, torts, and promissory estoppel.每个研究领域都有它自己的语言和思考方式。
数学家谈论定理、积分以及向量空间。
心理学家谈论自我、本能、以及认知的不一致性。
律师谈论犯罪地点、侵权行为以及约定的禁止翻供。
2.Economics is no different. Supply, demand, elasticity, comparative advantage, consumer surplus, deadweight loss—these terms are part of the economist’s language. In the co ming chapters, you will encounter many new terms and some familiar words that economists use in specialized ways. At first, this new language may seem needlessly arcane. But, as you will see, its value lies in its ability to provide you a new and useful way of thinking about the world in which you live.经济学家也一样。
离散数学中英文名词对照表
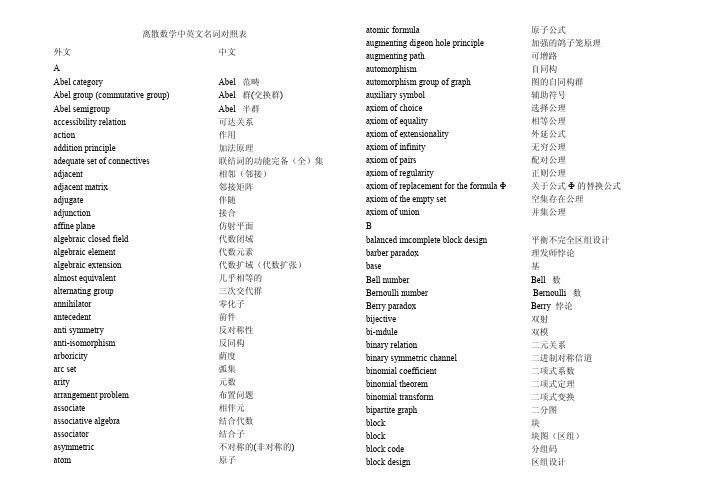
离散数学中英文名词对照表外文中文AAbel category Abel 范畴Abel group (commutative group) Abel 群(交换群)Abel semigroup Abel 半群accessibility relation 可达关系action 作用addition principle 加法原理adequate set of connectives 联结词的功能完备(全)集adjacent 相邻(邻接)adjacent matrix 邻接矩阵adjugate 伴随adjunction 接合affine plane 仿射平面algebraic closed field 代数闭域algebraic element 代数元素algebraic extension 代数扩域(代数扩张)almost equivalent 几乎相等的alternating group 三次交代群annihilator 零化子antecedent 前件anti symmetry 反对称性anti-isomorphism 反同构arboricity 荫度arc set 弧集arity 元数arrangement problem 布置问题associate 相伴元associative algebra 结合代数associator 结合子asymmetric 不对称的(非对称的)atom 原子atomic formula 原子公式augmenting digeon hole principle 加强的鸽子笼原理augmenting path 可增路automorphism 自同构automorphism group of graph 图的自同构群auxiliary symbol 辅助符号axiom of choice 选择公理axiom of equality 相等公理axiom of extensionality 外延公式axiom of infinity 无穷公理axiom of pairs 配对公理axiom of regularity 正则公理axiom of replacement for the formula Ф关于公式Ф的替换公式axiom of the empty set 空集存在公理axiom of union 并集公理Bbalanced imcomplete block design 平衡不完全区组设计barber paradox 理发师悖论base 基Bell number Bell 数Bernoulli number Bernoulli 数Berry paradox Berry 悖论bijective 双射bi-mdule 双模binary relation 二元关系binary symmetric channel 二进制对称信道binomial coefficient 二项式系数binomial theorem 二项式定理binomial transform 二项式变换bipartite graph 二分图block 块block 块图(区组)block code 分组码block design 区组设计Bondy theorem Bondy 定理Boole algebra Boole 代数Boole function Boole 函数Boole homomorophism Boole 同态Boole lattice Boole 格bound occurrence 约束出现bound variable 约束变量bounded lattice 有界格bridge 桥Bruijn theorem Bruijn 定理Burali-Forti paradox Burali-Forti 悖论Burnside lemma Burnside 引理Ccage 笼canonical epimorphism 标准满态射Cantor conjecture Cantor 猜想Cantor diagonal method Cantor 对角线法Cantor paradox Cantor 悖论cardinal number 基数Cartesion product of graph 图的笛卡儿积Catalan number Catalan 数category 范畴Cayley graph Cayley 图Cayley theorem Cayley 定理center 中心characteristic function 特征函数characteristic of ring 环的特征characteristic polynomial 特征多项式check digits 校验位Chinese postman problem 中国邮递员问题chromatic number 色数chromatic polynomial 色多项式circuit 回路circulant graph 循环图circumference 周长class 类classical completeness 古典完全的classical consistent 古典相容的clique 团clique number 团数closed term 闭项closure 闭包closure of graph 图的闭包code 码code element 码元code length 码长code rate 码率code word 码字coefficient 系数coimage 上象co-kernal 上核coloring 着色coloring problem 着色问题combination number 组合数combination with repetation 可重组合common factor 公因子commutative diagram 交换图commutative ring 交换环commutative seimgroup 交换半群complement 补图(子图的余) complement element 补元complemented lattice 有补格complete bipartite graph 完全二分图complete graph 完全图complete k-partite graph 完全k-分图complete lattice 完全格composite 复合composite operation 复合运算composition (molecular proposition) 复合(分子)命题composition of graph (lexicographic product)图的合成(字典积)concatenation (juxtaposition) 邻接运算concatenation graph 连通图congruence relation 同余关系conjunctive normal form 正则合取范式connected component 连通分支connective 连接的connectivity 连通度consequence 推论(后承)consistent (non-contradiction) 相容性(无矛盾性)continuum 连续统contraction of graph 图的收缩contradiction 矛盾式(永假式)contravariant functor 反变函子coproduct 上积corank 余秩correct error 纠正错误corresponding universal map 对应的通用映射countably infinite set 可列无限集(可列集)covariant functor (共变)函子covering 覆盖covering number 覆盖数Coxeter graph Coxeter 图crossing number of graph 图的叉数cuset 陪集cotree 余树cut edge 割边cut vertex 割点cycle 圈cycle basis 圈基cycle matrix 圈矩阵cycle rank 圈秩cycle space 圈空间cycle vector 圈向量cyclic group 循环群cyclic index 循环(轮转)指标cyclic monoid 循环单元半群cyclic permutation 圆圈排列cyclic semigroup 循环半群DDe Morgan law De Morgan 律decision procedure 判决过程decoding table 译码表deduction theorem 演绎定理degree 次数,次(度)degree sequence 次(度)序列derivation algebra 微分代数Descartes product Descartes 积designated truth value 特指真值detect errer 检验错误deterministic 确定的diagonal functor 对角线函子diameter 直径digraph 有向图dilemma 二难推理direct consequence 直接推论(直接后承)direct limit 正向极限direct sum 直和directed by inclution 被包含关系定向discrete Fourier transform 离散 Fourier 变换disjunctive normal form 正则析取范式disjunctive syllogism 选言三段论distance 距离distance transitive graph 距离传递图distinguished element 特异元distributive lattice 分配格divisibility 整除division subring 子除环divison ring 除环divisor (factor) 因子domain 定义域Driac condition Dirac 条件dual category 对偶范畴dual form 对偶式dual graph 对偶图dual principle 对偶原则(对偶原理) dual statement 对偶命题dummy variable 哑变量(哑变元)Eeccentricity 离心率edge chromatic number 边色数edge coloring 边着色edge connectivity 边连通度edge covering 边覆盖edge covering number 边覆盖数edge cut 边割集edge set 边集edge-independence number 边独立数eigenvalue of graph 图的特征值elementary divisor ideal 初等因子理想elementary product 初等积elementary sum 初等和empty graph 空图empty relation 空关系empty set 空集endomorphism 自同态endpoint 端点enumeration function 计数函数epimorphism 满态射equipotent 等势equivalent category 等价范畴equivalent class 等价类equivalent matrix 等价矩阵equivalent object 等价对象equivalent relation 等价关系error function 错误函数error pattern 错误模式Euclid algorithm 欧几里德算法Euclid domain 欧氏整环Euler characteristic Euler 特征Euler function Euler 函数Euler graph Euler 图Euler number Euler 数Euler polyhedron formula Euler 多面体公式Euler tour Euler 闭迹Euler trail Euler 迹existential generalization 存在推广规则existential quantifier 存在量词existential specification 存在特指规则extended Fibonacci number 广义 Fibonacci 数extended Lucas number 广义Lucas 数extension 扩充(扩张)extension field 扩域extension graph 扩图exterior algebra 外代数Fface 面factor 因子factorable 可因子化的factorization 因子分解faithful (full) functor 忠实(完满)函子Ferrers graph Ferrers 图Fibonacci number Fibonacci 数field 域filter 滤子finite extension 有限扩域finite field (Galois field ) 有限域(Galois 域)finite dimensional associative division algebra有限维结合可除代数finite set 有限(穷)集finitely generated module 有限生成模first order theory with equality 带符号的一阶系统five-color theorem 五色定理five-time-repetition 五倍重复码fixed point 不动点forest 森林forgetful functor 忘却函子four-color theorem(conjecture) 四色定理(猜想)F-reduced product F-归纳积free element 自由元free monoid 自由单元半群free occurrence 自由出现free R-module 自由R-模free variable 自由变元free-Ω-algebra 自由Ω代数function scheme 映射格式GGalileo paradox Galileo 悖论Gauss coefficient Gauss 系数GBN (Gödel-Bernays-von Neumann system)GBN系统generalized petersen graph 广义 petersen 图generating function 生成函数generating procedure 生成过程generator 生成子(生成元)generator matrix 生成矩阵genus 亏格girth (腰)围长Gödel completeness theorem Gödel 完全性定理golden section number 黄金分割数(黄金分割率)graceful graph 优美图graceful tree conjecture 优美树猜想graph 图graph of first class for edge coloring 第一类边色图graph of second class for edge coloring 第二类边色图graph rank 图秩graph sequence 图序列greatest common factor 最大公因子greatest element 最大元(素)Grelling paradox Grelling 悖论Grötzsch graph Grötzsch 图group 群group code 群码group of graph 图的群HHajós conjecture Hajós 猜想Hamilton cycle Hamilton 圈Hamilton graph Hamilton 图Hamilton path Hamilton 路Harary graph Harary 图Hasse graph Hasse 图Heawood graph Heawood 图Herschel graph Herschel 图hom functor hom 函子homemorphism 图的同胚homomorphism 同态(同态映射)homomorphism of graph 图的同态hyperoctahedron 超八面体图hypothelical syllogism 假言三段论hypothese (premise) 假设(前提)Iideal 理想identity 单位元identity natural transformation 恒等自然变换imbedding 嵌入immediate predcessor 直接先行immediate successor 直接后继incident 关联incident axiom 关联公理incident matrix 关联矩阵inclusion and exclusion principle 包含与排斥原理inclusion relation 包含关系indegree 入次(入度)independent 独立的independent number 独立数independent set 独立集independent transcendental element 独立超越元素index 指数individual variable 个体变元induced subgraph 导出子图infinite extension 无限扩域infinite group 无限群infinite set 无限(穷)集initial endpoint 始端initial object 初始对象injection 单射injection functor 单射函子injective (one to one mapping) 单射(内射)inner face 内面inner neighbour set 内(入)邻集integral domain 整环integral subdomain 子整环internal direct sum 内直和intersection 交集intersection of graph 图的交intersection operation 交运算interval 区间invariant factor 不变因子invariant factor ideal 不变因子理想inverse limit 逆向极限inverse morphism 逆态射inverse natural transformation 逆自然变换inverse operation 逆运算inverse relation 逆关系inversion 反演isomorphic category 同构范畴isomorphism 同构态射isomorphism of graph 图的同构join of graph 图的联JJordan algebra Jordan 代数Jordan product (anti-commutator) Jordan乘积(反交换子)Jordan sieve formula Jordan 筛法公式j-skew j-斜元juxtaposition 邻接乘法Kk-chromatic graph k-色图k-connected graph k-连通图k-critical graph k-色临界图k-edge chromatic graph k-边色图k-edge-connected graph k-边连通图k-edge-critical graph k-边临界图kernel 核Kirkman schoolgirl problem Kirkman 女生问题Kuratowski theorem Kuratowski 定理Llabeled graph 有标号图Lah number Lah 数Latin rectangle Latin 矩形Latin square Latin 方lattice 格lattice homomorphism 格同态law 规律leader cuset 陪集头least element 最小元least upper bound 上确界(最小上界)left (right) identity 左(右)单位元left (right) invertible element 左(右)可逆元left (right) module 左(右)模left (right) zero 左(右)零元left (right) zero divisor 左(右)零因子left adjoint functor 左伴随函子left cancellable 左可消的left coset 左陪集length 长度Lie algebra Lie 代数line- group 图的线群logically equivanlent 逻辑等价logically implies 逻辑蕴涵logically valid 逻辑有效的(普效的)loop 环Lucas number Lucas 数Mmagic 幻方many valued proposition logic 多值命题逻辑matching 匹配mathematical structure 数学结构matrix representation 矩阵表示maximal element 极大元maximal ideal 极大理想maximal outerplanar graph 极大外平面图maximal planar graph 极大平面图maximum matching 最大匹配maxterm 极大项(基本析取式)maxterm normal form(conjunctive normal form) 极大项范式(合取范式)McGee graph McGee 图meet 交Menger theorem Menger 定理Meredith graph Meredith 图message word 信息字mini term 极小项minimal κ-connected graph 极小κ-连通图minimal polynomial 极小多项式Minimanoff paradox Minimanoff 悖论minimum distance 最小距离Minkowski sum Minkowski 和minterm (fundamental conjunctive form) 极小项(基本合取式)minterm normal form(disjunctive normal form)极小项范式(析取范式)Möbius function Möbius 函数Möbius ladder Möbius 梯Möbius transform (inversion) Möbius 变换(反演)modal logic 模态逻辑model 模型module homomorphism 模同态(R-同态)modus ponens 分离规则modus tollens 否定后件式module isomorphism 模同构monic morphism 单同态monoid 单元半群monomorphism 单态射morphism (arrow) 态射(箭)Möbius function Möbius 函数Möbius ladder Möbius 梯Möbius transform (inversion) Möbius 变换(反演)multigraph 多重图multinomial coefficient 多项式系数multinomial expansion theorem 多项式展开定理multiple-error-correcting code 纠多错码multiplication principle 乘法原理mutually orthogonal Latin square 相互正交拉丁方Nn-ary operation n-元运算n-ary product n-元积natural deduction system 自然推理系统natural isomorphism 自然同构natural transformation 自然变换neighbour set 邻集next state 下一个状态next state transition function 状态转移函数non-associative algebra 非结合代数non-standard logic 非标准逻辑Norlund formula Norlund 公式normal form 正规形normal model 标准模型normal subgroup (invariant subgroup) 正规子群(不变子群)n-relation n-元关系null object 零对象nullary operation 零元运算Oobject 对象orbit 轨道order 阶order ideal 阶理想Ore condition Ore 条件orientation 定向orthogonal Latin square 正交拉丁方orthogonal layout 正交表outarc 出弧outdegree 出次(出度)outer face 外面outer neighbour 外(出)邻集outerneighbour set 出(外)邻集outerplanar graph 外平面图Ppancycle graph 泛圈图parallelism 平行parallelism class 平行类parity-check code 奇偶校验码parity-check equation 奇偶校验方程parity-check machine 奇偶校验器parity-check matrix 奇偶校验矩阵partial function 偏函数partial ordering (partial relation) 偏序关系partial order relation 偏序关系partial order set (poset) 偏序集partition 划分,分划,分拆partition number of integer 整数的分拆数partition number of set 集合的划分数Pascal formula Pascal 公式path 路perfect code 完全码perfect t-error-correcting code 完全纠-错码perfect graph 完美图permutation 排列(置换)permutation group 置换群permutation with repetation 可重排列Petersen graph Petersen 图p-graph p-图Pierce arrow Pierce 箭pigeonhole principle 鸽子笼原理planar graph (可)平面图plane graph 平面图Pólya theorem Pólya 定理polynomail 多项式polynomial code 多项式码polynomial representation 多项式表示法polynomial ring 多项式环possible world 可能世界power functor 幂函子power of graph 图的幂power set 幂集predicate 谓词prenex normal form 前束范式pre-ordered set 拟序集primary cycle module 准素循环模prime field 素域prime to each other 互素primitive connective 初始联结词primitive element 本原元primitive polynomial 本原多项式principal ideal 主理想principal ideal domain 主理想整环principal of duality 对偶原理principal of redundancy 冗余性原则product 积product category 积范畴product-sum form 积和式proof (deduction) 证明(演绎)proper coloring 正常着色proper factor 真正因子proper filter 真滤子proper subgroup 真子群properly inclusive relation 真包含关系proposition 命题propositional constant 命题常量propositional formula(well-formed formula,wff)命题形式(合式公式)propositional function 命题函数propositional variable 命题变量pullback 拉回(回拖) pushout 推出Qquantification theory 量词理论quantifier 量词quasi order relation 拟序关系quaternion 四元数quotient (difference) algebra 商(差)代数quotient algebra 商代数quotient field (field of fraction) 商域(分式域)quotient group 商群quotient module 商模quotient ring (difference ring , residue ring) 商环(差环,同余类环)quotient set 商集RRamsey graph Ramsey 图Ramsey number Ramsey 数Ramsey theorem Ramsey 定理range 值域rank 秩reconstruction conjecture 重构猜想redundant digits 冗余位reflexive 自反的regular graph 正则图regular representation 正则表示relation matrix 关系矩阵replacement theorem 替换定理representation 表示representation functor 可表示函子restricted proposition form 受限命题形式restriction 限制retraction 收缩Richard paradox Richard 悖论right adjoint functor 右伴随函子right cancellable 右可消的right factor 右因子right zero divison 右零因子ring 环ring of endomorphism 自同态环ring with unity element 有单元的环R-linear independence R-线性无关root field 根域rule of inference 推理规则Russell paradox Russell 悖论Ssatisfiable 可满足的saturated 饱和的scope 辖域section 截口self-complement graph 自补图semantical completeness 语义完全的(弱完全的)semantical consistent 语义相容semigroup 半群separable element 可分元separable extension 可分扩域sequent 矢列式sequential 序列的Sheffer stroke Sheffer 竖(谢弗竖)simple algebraic extension 单代数扩域simple extension 单扩域simple graph 简单图simple proposition (atomic proposition) 简单(原子)命题simple transcental extension 单超越扩域simplication 简化规则slope 斜率small category 小范畴smallest element 最小元(素)Socrates argument Socrates 论断(苏格拉底论断)soundness (validity) theorem 可靠性(有效性)定理spanning subgraph 生成子图spanning tree 生成树spectra of graph 图的谱spetral radius 谱半径splitting field 分裂域standard model 标准模型standard monomil 标准单项式Steiner triple Steiner 三元系大集Stirling number Stirling 数Stirling transform Stirling 变换subalgebra 子代数subcategory 子范畴subdirect product 子直积subdivison of graph 图的细分subfield 子域subformula 子公式subdivision of graph 图的细分subgraph 子图subgroup 子群sub-module 子模subrelation 子关系subring 子环sub-semigroup 子半群subset 子集substitution theorem 代入定理substraction 差集substraction operation 差运算succedent 后件surjection (surjective) 满射switching-network 开关网络Sylvester formula Sylvester公式symmetric 对称的symmetric difference 对称差symmetric graph 对称图symmetric group 对称群syndrome 校验子syntactical completeness 语法完全的(强完全的)Syntactical consistent 语法相容system Ł3 , Łn , Łא0 , Łא系统Ł3 , Łn , Łא0 , Łאsystem L 公理系统 Lsystem Ł公理系统Łsystem L1 公理系统 L1system L2 公理系统 L2system L3 公理系统 L3system L4 公理系统 L4system L5 公理系统 L5system L6 公理系统 L6system Łn 公理系统Łnsystem of modal prepositional logic 模态命题逻辑系统system Pm 系统 Pmsystem S1 公理系统 S1system T (system M) 公理系统 T(系统M)Ttautology 重言式(永真公式)technique of truth table 真值表技术term 项terminal endpoint 终端terminal object 终结对象t-error-correcing BCH code 纠 t -错BCH码theorem (provable formal) 定理(可证公式)thickess 厚度timed sequence 时间序列torsion 扭元torsion module 扭模total chromatic number 全色数total chromatic number conjecture 全色数猜想total coloring 全着色total graph 全图total matrix ring 全方阵环total order set 全序集total permutation 全排列total relation 全关系tournament 竞赛图trace (trail) 迹tranformation group 变换群transcendental element 超越元素transitive 传递的tranverse design 横截设计traveling saleman problem 旅行商问题tree 树triple system 三元系triple-repetition code 三倍重复码trivial graph 平凡图trivial subgroup 平凡子群true in an interpretation 解释真truth table 真值表truth value function 真值函数Turán graph Turán 图Turán theorem Turán 定理Tutte graph Tutte 图Tutte theorem Tutte 定理Tutte-coxeter graph Tutte-coxeter 图UUlam conjecture Ulam 猜想ultrafilter 超滤子ultrapower 超幂ultraproduct 超积unary operation 一元运算unary relation 一元关系underlying graph 基础图undesignated truth value 非特指值undirected graph 无向图union 并(并集)union of graph 图的并union operation 并运算unique factorization 唯一分解unique factorization domain (Gauss domain) 唯一分解整域unique k-colorable graph 唯一k着色unit ideal 单位理想unity element 单元universal 全集universal algebra 泛代数(Ω代数)universal closure 全称闭包universal construction 通用结构universal enveloping algebra 通用包络代数universal generalization 全称推广规则universal quantifier 全称量词universal specification 全称特指规则universal upper bound 泛上界unlabeled graph 无标号图untorsion 无扭模upper (lower) bound 上(下)界useful equivalent 常用等值式useless code 废码字Vvalence 价valuation 赋值Vandermonde formula Vandermonde 公式variery 簇Venn graph Venn 图vertex cover 点覆盖vertex set 点割集vertex transitive graph 点传递图Vizing theorem Vizing 定理Wwalk 通道weakly antisymmetric 弱反对称的weight 重(权)weighted form for Burnside lemma 带权形式的Burnside引理well-formed formula (wff) 合式公式(wff)word 字Zzero divison 零因子zero element (universal lower bound) 零元(泛下界)ZFC (Zermelo-Fraenkel-Cohen) system ZFC系统form)normal(Skolemformnormalprenex-存在正则前束范式(Skolem 正则范式)3-value proposition logic 三值命题逻辑。
the myth of sisyphus西西弗斯的神话

Below is given annual work summary, do not need friends can download after editor deleted!!!!!! Welcome to visit againXXXX annual work summaryDear every leader, colleagues:Look back end of XXXX, XXXX years of work, have the joy of success in your work, have a collaboration with colleagues, working hard, also have disappointed when encountered difficulties and setbacks. Imperceptible in tense and orderly to be over a year, a year, under the loving care and guidance of the leadership of the company, under the support and help of colleagues, through their own efforts, various aspects have made certain progress, better to complete the job. For better work, sum up experience and lessons, will now work a brief summary.To continuously strengthen learning, improve their comprehensive quality. With good comprehensive quality is the precondition of completes the labor of duty and conditions. A year always put learning in the important position, trying to improve their comprehensive quality. Continuous learning professional skills, learn from surrounding colleagues with rich work experience, equip themselves with knowledge, the expanded aspect of knowledge, efforts to improve their comprehensive quality.The second Do best, strictly perform their responsibilities. Set up the company, to maximize the customer to the satisfaction of the company's products, do a good job in technical services and product promotion to the company. And collected on the properties of the products of the company, in order to make improvement in time, make the products better meet the using demand of the scene.Three to learn to be good at communication, coordinating assistance. On‐site technical service personnel should not only have strong professional technology, should also have good communication ability, a lot of a product due to improper operation to appear problem, but often not customers reflect the quality of no, so this time we need to find out the crux, and customer communication, standardized operation, to avoid customer's mistrust of the products and even the damage of the company's image. Some experiences in the past work, mentality is very important in the work, work to have passion, keep the smile of sunshine, can close the distance between people, easy to communicate with the customer. Do better in the daily work to communicate with customers and achieve customer satisfaction, excellent technical service every time, on behalf of the customer on our products much a understanding and trust.Fourth, we need to continue to learn professional knowledge, do practical grasp skilled operation. Over the past year, through continuous learning and fumble, studied the gas generation, collection and methods, gradually familiar with and master the company introduced the working principle, operation method of gas machine. With the help of the department leaders and colleagues, familiar with and master the launch of the division principle, debugging method of the control system, and to wuhan Chen Guchong garbage power plant of gas machine control system transformation, learn to debug, accumulated some experience. All in all, over the past year, did some work, have also made some achievements, but the results can only represent the past, there are some problems to work, can't meet the higher requirements. In the future work, I must develop the oneself advantage, lack of correct, foster strengths and circumvent weaknesses, for greater achievements. Looking forward to XXXX years of work, I'll be more efforts, constant progress in their jobs, make greater achievements. Every year I have progress, the growth of believe will get greater returns, I will my biggest contribution to the development of the company, believe in yourself do better next year!I wish you all work study progress in the year to come.。
专八英语阅读

英语专业八级考试TEM-8阅读理解练习册(1)(英语专业2012级)UNIT 1Text AEvery minute of every day, what ecologist生态学家James Carlton calls a global ―conveyor belt‖, redistributes ocean organisms生物.It’s planetwide biological disruption生物的破坏that scientists have barely begun to understand.Dr. Carlton —an oceanographer at Williams College in Williamstown,Mass.—explains that, at any given moment, ―There are several thousand marine species traveling… in the ballast water of ships.‖ These creatures move from coastal waters where they fit into the local web of life to places where some of them could tear that web apart. This is the larger dimension of the infamous无耻的,邪恶的invasion of fish-destroying, pipe-clogging zebra mussels有斑马纹的贻贝.Such voracious贪婪的invaders at least make their presence known. What concerns Carlton and his fellow marine ecologists is the lack of knowledge about the hundreds of alien invaders that quietly enter coastal waters around the world every day. Many of them probably just die out. Some benignly亲切地,仁慈地—or even beneficially — join the local scene. But some will make trouble.In one sense, this is an old story. Organisms have ridden ships for centuries. They have clung to hulls and come along with cargo. What’s new is the scale and speed of the migrations made possible by the massive volume of ship-ballast water压载水— taken in to provide ship stability—continuously moving around the world…Ships load up with ballast water and its inhabitants in coastal waters of one port and dump the ballast in another port that may be thousands of kilometers away. A single load can run to hundreds of gallons. Some larger ships take on as much as 40 million gallons. The creatures that come along tend to be in their larva free-floating stage. When discharged排出in alien waters they can mature into crabs, jellyfish水母, slugs鼻涕虫,蛞蝓, and many other forms.Since the problem involves coastal species, simply banning ballast dumps in coastal waters would, in theory, solve it. Coastal organisms in ballast water that is flushed into midocean would not survive. Such a ban has worked for North American Inland Waterway. But it would be hard to enforce it worldwide. Heating ballast water or straining it should also halt the species spread. But before any such worldwide regulations were imposed, scientists would need a clearer view of what is going on.The continuous shuffling洗牌of marine organisms has changed the biology of the sea on a global scale. It can have devastating effects as in the case of the American comb jellyfish that recently invaded the Black Sea. It has destroyed that sea’s anchovy鳀鱼fishery by eating anchovy eggs. It may soon spread to western and northern European waters.The maritime nations that created the biological ―conveyor belt‖ should support a coordinated international effort to find out what is going on and what should be done about it. (456 words)1.According to Dr. Carlton, ocean organism‟s are_______.A.being moved to new environmentsB.destroying the planetC.succumbing to the zebra musselD.developing alien characteristics2.Oceanographers海洋学家are concerned because_________.A.their knowledge of this phenomenon is limitedB.they believe the oceans are dyingC.they fear an invasion from outer-spaceD.they have identified thousands of alien webs3.According to marine ecologists, transplanted marinespecies____________.A.may upset the ecosystems of coastal watersB.are all compatible with one anotherC.can only survive in their home watersD.sometimes disrupt shipping lanes4.The identified cause of the problem is_______.A.the rapidity with which larvae matureB. a common practice of the shipping industryC. a centuries old speciesD.the world wide movement of ocean currents5.The article suggests that a solution to the problem__________.A.is unlikely to be identifiedB.must precede further researchC.is hypothetically假设地,假想地easyD.will limit global shippingText BNew …Endangered‟ List Targets Many US RiversIt is hard to think of a major natural resource or pollution issue in North America today that does not affect rivers.Farm chemical runoff残渣, industrial waste, urban storm sewers, sewage treatment, mining, logging, grazing放牧,military bases, residential and business development, hydropower水力发电,loss of wetlands. The list goes on.Legislation like the Clean Water Act and Wild and Scenic Rivers Act have provided some protection, but threats continue.The Environmental Protection Agency (EPA) reported yesterday that an assessment of 642,000 miles of rivers and streams showed 34 percent in less than good condition. In a major study of the Clean Water Act, the Natural Resources Defense Council last fall reported that poison runoff impairs损害more than 125,000 miles of rivers.More recently, the NRDC and Izaak Walton League warned that pollution and loss of wetlands—made worse by last year’s flooding—is degrading恶化the Mississippi River ecosystem.On Tuesday, the conservation group保护组织American Rivers issued its annual list of 10 ―endangered‖ and 20 ―threatened‖ rivers in 32 states, the District of Colombia, and Canada.At the top of the list is the Clarks Fork of the Yellowstone River, whereCanadian mining firms plan to build a 74-acre英亩reservoir水库,蓄水池as part of a gold mine less than three miles from Yellowstone National Park. The reservoir would hold the runoff from the sulfuric acid 硫酸used to extract gold from crushed rock.―In the event this tailings pond failed, the impact to th e greater Yellowstone ecosystem would be cataclysmic大变动的,灾难性的and the damage irreversible不可逆转的.‖ Sen. Max Baucus of Montana, chairman of the Environment and Public Works Committee, wrote to Noranda Minerals Inc., an owner of the ― New World Mine‖.Last fall, an EPA official expressed concern about the mine and its potential impact, especially the plastic-lined storage reservoir. ― I am unaware of any studies evaluating how a tailings pond尾矿池,残渣池could be maintained to ensure its structural integrity forev er,‖ said Stephen Hoffman, chief of the EPA’s Mining Waste Section. ―It is my opinion that underwater disposal of tailings at New World may present a potentially significant threat to human health and the environment.‖The results of an environmental-impact statement, now being drafted by the Forest Service and Montana Department of State Lands, could determine the mine’s future…In its recent proposal to reauthorize the Clean Water Act, the Clinton administration noted ―dramatically improved water quality since 1972,‖ when the act was passed. But it also reported that 30 percent of riverscontinue to be degraded, mainly by silt泥沙and nutrients from farm and urban runoff, combined sewer overflows, and municipal sewage城市污水. Bottom sediments沉积物are contaminated污染in more than 1,000 waterways, the administration reported in releasing its proposal in January. Between 60 and 80 percent of riparian corridors (riverbank lands) have been degraded.As with endangered species and their habitats in forests and deserts, the complexity of ecosystems is seen in rivers and the effects of development----beyond the obvious threats of industrial pollution, municipal waste, and in-stream diversions改道to slake消除the thirst of new communities in dry regions like the Southwes t…While there are many political hurdles障碍ahead, reauthorization of the Clean Water Act this year holds promise for US rivers. Rep. Norm Mineta of California, who chairs the House Committee overseeing the bill, calls it ―probably the most important env ironmental legislation this Congress will enact.‖ (553 words)6.According to the passage, the Clean Water Act______.A.has been ineffectiveB.will definitely be renewedC.has never been evaluatedD.was enacted some 30 years ago7.“Endangered” rivers are _________.A.catalogued annuallyB.less polluted than ―threatened rivers‖C.caused by floodingD.adjacent to large cities8.The “cataclysmic” event referred to in paragraph eight would be__________.A. fortuitous偶然的,意外的B. adventitious外加的,偶然的C. catastrophicD. precarious不稳定的,危险的9. The owners of the New World Mine appear to be______.A. ecologically aware of the impact of miningB. determined to construct a safe tailings pondC. indifferent to the concerns voiced by the EPAD. willing to relocate operations10. The passage conveys the impression that_______.A. Canadians are disinterested in natural resourcesB. private and public environmental groups aboundC. river banks are erodingD. the majority of US rivers are in poor conditionText CA classic series of experiments to determine the effects ofoverpopulation on communities of rats was reported in February of 1962 in an article in Scientific American. The experiments were conducted by a psychologist, John B. Calhoun and his associates. In each of these experiments, an equal number of male and female adult rats were placed in an enclosure and given an adequate supply of food, water, and other necessities. The rat populations were allowed to increase. Calhoun knew from experience approximately how many rats could live in the enclosures without experiencing stress due to overcrowding. He allowed the population to increase to approximately twice this number. Then he stabilized the population by removing offspring that were not dependent on their mothers. He and his associates then carefully observed and recorded behavior in these overpopulated communities. At the end of their experiments, Calhoun and his associates were able to conclude that overcrowding causes a breakdown in the normal social relationships among rats, a kind of social disease. The rats in the experiments did not follow the same patterns of behavior as rats would in a community without overcrowding.The females in the rat population were the most seriously affected by the high population density: They showed deviant异常的maternal behavior; they did not behave as mother rats normally do. In fact, many of the pups幼兽,幼崽, as rat babies are called, died as a result of poor maternal care. For example, mothers sometimes abandoned their pups,and, without their mothers' care, the pups died. Under normal conditions, a mother rat would not leave her pups alone to die. However, the experiments verified that in overpopulated communities, mother rats do not behave normally. Their behavior may be considered pathologically 病理上,病理学地diseased.The dominant males in the rat population were the least affected by overpopulation. Each of these strong males claimed an area of the enclosure as his own. Therefore, these individuals did not experience the overcrowding in the same way as the other rats did. The fact that the dominant males had adequate space in which to live may explain why they were not as seriously affected by overpopulation as the other rats. However, dominant males did behave pathologically at times. Their antisocial behavior consisted of attacks on weaker male,female, and immature rats. This deviant behavior showed that even though the dominant males had enough living space, they too were affected by the general overcrowding in the enclosure.Non-dominant males in the experimental rat communities also exhibited deviant social behavior. Some withdrew completely; they moved very little and ate and drank at times when the other rats were sleeping in order to avoid contact with them. Other non-dominant males were hyperactive; they were much more active than is normal, chasing other rats and fighting each other. This segment of the rat population, likeall the other parts, was affected by the overpopulation.The behavior of the non-dominant males and of the other components of the rat population has parallels in human behavior. People in densely populated areas exhibit deviant behavior similar to that of the rats in Calhoun's experiments. In large urban areas such as New York City, London, Mexican City, and Cairo, there are abandoned children. There are cruel, powerful individuals, both men and women. There are also people who withdraw and people who become hyperactive. The quantity of other forms of social pathology such as murder, rape, and robbery also frequently occur in densely populated human communities. Is the principal cause of these disorders overpopulation? Calhoun’s experiments suggest that it might be. In any case, social scientists and city planners have been influenced by the results of this series of experiments.11. Paragraph l is organized according to__________.A. reasonsB. descriptionC. examplesD. definition12.Calhoun stabilized the rat population_________.A. when it was double the number that could live in the enclosure without stressB. by removing young ratsC. at a constant number of adult rats in the enclosureD. all of the above are correct13.W hich of the following inferences CANNOT be made from theinformation inPara. 1?A. Calhoun's experiment is still considered important today.B. Overpopulation causes pathological behavior in rat populations.C. Stress does not occur in rat communities unless there is overcrowding.D. Calhoun had experimented with rats before.14. Which of the following behavior didn‟t happen in this experiment?A. All the male rats exhibited pathological behavior.B. Mother rats abandoned their pups.C. Female rats showed deviant maternal behavior.D. Mother rats left their rat babies alone.15. The main idea of the paragraph three is that __________.A. dominant males had adequate living spaceB. dominant males were not as seriously affected by overcrowding as the otherratsC. dominant males attacked weaker ratsD. the strongest males are always able to adapt to bad conditionsText DThe first mention of slavery in the statutes法令,法规of the English colonies of North America does not occur until after 1660—some forty years after the importation of the first Black people. Lest we think that existed in fact before it did in law, Oscar and Mary Handlin assure us, that the status of B lack people down to the 1660’s was that of servants. A critique批判of the Handlins’ interpretation of why legal slavery did not appear until the 1660’s suggests that assumptions about the relation between slavery and racial prejudice should be reexamined, and that explanation for the different treatment of Black slaves in North and South America should be expanded.The Handlins explain the appearance of legal slavery by arguing that, during the 1660’s, the position of white servants was improving relative to that of black servants. Thus, the Handlins contend, Black and White servants, heretofore treated alike, each attained a different status. There are, however, important objections to this argument. First, the Handlins cannot adequately demonstrate that t he White servant’s position was improving, during and after the 1660’s; several acts of the Maryland and Virginia legislatures indicate otherwise. Another flaw in the Handlins’ interpretation is their assumption that prior to the establishment of legal slavery there was no discrimination against Black people. It is true that before the 1660’s Black people were rarely called slaves. But this shouldnot overshadow evidence from the 1630’s on that points to racial discrimination without using the term slavery. Such discrimination sometimes stopped short of lifetime servitude or inherited status—the two attributes of true slavery—yet in other cases it included both. The Handlins’ argument excludes the real possibility that Black people in the English colonies were never treated as the equals of White people.The possibility has important ramifications后果,影响.If from the outset Black people were discriminated against, then legal slavery should be viewed as a reflection and an extension of racial prejudice rather than, as many historians including the Handlins have argued, the cause of prejudice. In addition, the existence of discrimination before the advent of legal slavery offers a further explanation for the harsher treatment of Black slaves in North than in South America. Freyre and Tannenbaum have rightly argued that the lack of certain traditions in North America—such as a Roman conception of slavery and a Roman Catholic emphasis on equality— explains why the treatment of Black slaves was more severe there than in the Spanish and Portuguese colonies of South America. But this cannot be the whole explanation since it is merely negative, based only on a lack of something. A more compelling令人信服的explanation is that the early and sometimes extreme racial discrimination in the English colonies helped determine the particular nature of the slavery that followed. (462 words)16. Which of the following is the most logical inference to be drawn from the passage about the effects of “several acts of the Maryland and Virginia legislatures” (Para.2) passed during and after the 1660‟s?A. The acts negatively affected the pre-1660’s position of Black as wellas of White servants.B. The acts had the effect of impairing rather than improving theposition of White servants relative to what it had been before the 1660’s.C. The acts had a different effect on the position of white servants thandid many of the acts passed during this time by the legislatures of other colonies.D. The acts, at the very least, caused the position of White servants toremain no better than it had been before the 1660’s.17. With which of the following statements regarding the status ofBlack people in the English colonies of North America before the 1660‟s would the author be LEAST likely to agree?A. Although black people were not legally considered to be slaves,they were often called slaves.B. Although subject to some discrimination, black people had a higherlegal status than they did after the 1660’s.C. Although sometimes subject to lifetime servitude, black peoplewere not legally considered to be slaves.D. Although often not treated the same as White people, black people,like many white people, possessed the legal status of servants.18. According to the passage, the Handlins have argued which of thefollowing about the relationship between racial prejudice and the institution of legal slavery in the English colonies of North America?A. Racial prejudice and the institution of slavery arose simultaneously.B. Racial prejudice most often the form of the imposition of inheritedstatus, one of the attributes of slavery.C. The source of racial prejudice was the institution of slavery.D. Because of the influence of the Roman Catholic Church, racialprejudice sometimes did not result in slavery.19. The passage suggests that the existence of a Roman conception ofslavery in Spanish and Portuguese colonies had the effect of _________.A. extending rather than causing racial prejudice in these coloniesB. hastening the legalization of slavery in these colonies.C. mitigating some of the conditions of slavery for black people in these coloniesD. delaying the introduction of slavery into the English colonies20. The author considers the explanation put forward by Freyre andTannenbaum for the treatment accorded B lack slaves in the English colonies of North America to be _____________.A. ambitious but misguidedB. valid有根据的but limitedC. popular but suspectD. anachronistic过时的,时代错误的and controversialUNIT 2Text AThe sea lay like an unbroken mirror all around the pine-girt, lonely shores of Orr’s Island. Tall, kingly spruce s wore their regal王室的crowns of cones high in air, sparkling with diamonds of clear exuded gum流出的树胶; vast old hemlocks铁杉of primeval原始的growth stood darkling in their forest shadows, their branches hung with long hoary moss久远的青苔;while feathery larches羽毛般的落叶松,turned to brilliant gold by autumn frosts, lighted up the darker shadows of the evergreens. It was one of those hazy朦胧的, calm, dissolving days of Indian summer, when everything is so quiet that the fainest kiss of the wave on the beach can be heard, and white clouds seem to faint into the blue of the sky, and soft swathing一长条bands of violet vapor make all earth look dreamy, and give to the sharp, clear-cut outlines of the northern landscape all those mysteries of light and shade which impart such tenderness to Italian scenery.The funeral was over,--- the tread鞋底的花纹/ 踏of many feet, bearing the heavy burden of two broken lives, had been to the lonely graveyard, and had come back again,--- each footstep lighter and more unconstrained不受拘束的as each one went his way from the great old tragedy of Death to the common cheerful of Life.The solemn black clock stood swaying with its eternal ―tick-tock, tick-tock,‖ in the kitchen of the brown house on Orr’s Island. There was there that sense of a stillness that can be felt,---such as settles down on a dwelling住处when any of its inmates have passed through its doors for the last time, to go whence they shall not return. The best room was shut up and darkened, with only so much light as could fall through a little heart-shaped hole in the window-shutter,---for except on solemn visits, or prayer-meetings or weddings, or funerals, that room formed no part of the daily family scenery.The kitchen was clean and ample, hearth灶台, and oven on one side, and rows of old-fashioned splint-bottomed chairs against the wall. A table scoured to snowy whiteness, and a little work-stand whereon lay the Bible, the Missionary Herald, and the Weekly Christian Mirror, before named, formed the principal furniture. One feature, however, must not be forgotten, ---a great sea-chest水手用的储物箱,which had been the companion of Zephaniah through all the countries of the earth. Old, and battered破旧的,磨损的, and unsightly难看的it looked, yet report said that there was good store within which men for the most part respect more than anything else; and, indeed it proved often when a deed of grace was to be done--- when a woman was suddenly made a widow in a coast gale大风,狂风, or a fishing-smack小渔船was run down in the fogs off the banks, leaving in some neighboring cottage a family of orphans,---in all such cases, the opening of this sea-chest was an event of good omen 预兆to the bereaved丧亲者;for Zephaniah had a large heart and a large hand, and was apt有…的倾向to take it out full of silver dollars when once it went in. So the ark of the covenant约柜could not have been looked on with more reverence崇敬than the neighbours usually showed to Captain Pennel’s sea-chest.1. The author describes Orr‟s Island in a(n)______way.A.emotionally appealing, imaginativeB.rational, logically preciseC.factually detailed, objectiveD.vague, uncertain2.According to the passage, the “best room”_____.A.has its many windows boarded upB.has had the furniture removedC.is used only on formal and ceremonious occasionsD.is the busiest room in the house3.From the description of the kitchen we can infer that thehouse belongs to people who_____.A.never have guestsB.like modern appliancesC.are probably religiousD.dislike housework4.The passage implies that_______.A.few people attended the funeralB.fishing is a secure vocationC.the island is densely populatedD.the house belonged to the deceased5.From the description of Zephaniah we can see thathe_________.A.was physically a very big manB.preferred the lonely life of a sailorC.always stayed at homeD.was frugal and saved a lotText BBasic to any understanding of Canada in the 20 years after the Second World War is the country' s impressive population growth. For every three Canadians in 1945, there were over five in 1966. In September 1966 Canada's population passed the 20 million mark. Most of this surging growth came from natural increase. The depression of the 1930s and the war had held back marriages, and the catching-up process began after 1945. The baby boom continued through the decade of the 1950s, producing a population increase of nearly fifteen percent in the five years from 1951 to 1956. This rate of increase had been exceeded only once before in Canada's history, in the decade before 1911 when the prairies were being settled. Undoubtedly, the good economic conditions of the 1950s supported a growth in the population, but the expansion also derived from a trend toward earlier marriages and an increase in the average size of families; In 1957 the Canadian birth rate stood at 28 per thousand, one of the highest in the world. After the peak year of 1957, thebirth rate in Canada began to decline. It continued falling until in 1966 it stood at the lowest level in 25 years. Partly this decline reflected the low level of births during the depression and the war, but it was also caused by changes in Canadian society. Young people were staying at school longer, more women were working; young married couples were buying automobiles or houses before starting families; rising living standards were cutting down the size of families. It appeared that Canada was once more falling in step with the trend toward smaller families that had occurred all through theWestern world since the time of the Industrial Revolution. Although the growth in Canada’s population had slowed down by 1966 (the cent), another increase in the first half of the 1960s was only nine percent), another large population wave was coming over the horizon. It would be composed of the children of the children who were born during the period of the high birth rate prior to 1957.6. What does the passage mainly discuss?A. Educational changes in Canadian society.B. Canada during the Second World War.C. Population trends in postwar Canada.D. Standards of living in Canada.7. According to the passage, when did Canada's baby boom begin?A. In the decade after 1911.B. After 1945.C. During the depression of the 1930s.D. In 1966.8. The author suggests that in Canada during the 1950s____________.A. the urban population decreased rapidlyB. fewer people marriedC. economic conditions were poorD. the birth rate was very high9. When was the birth rate in Canada at its lowest postwar level?A. 1966.B. 1957.C. 1956.D. 1951.10. The author mentions all of the following as causes of declines inpopulation growth after 1957 EXCEPT_________________.A. people being better educatedB. people getting married earlierC. better standards of livingD. couples buying houses11.I t can be inferred from the passage that before the IndustrialRevolution_______________.A. families were largerB. population statistics were unreliableC. the population grew steadilyD. economic conditions were badText CI was just a boy when my father brought me to Harlem for the first time, almost 50 years ago. We stayed at the hotel Theresa, a grand brick structure at 125th Street and Seventh avenue. Once, in the hotel restaurant, my father pointed out Joe Louis. He even got Mr. Brown, the hotel manager, to introduce me to him, a bit punchy强力的but still champ焦急as fast as I was concerned.Much has changed since then. Business and real estate are booming. Some say a new renaissance is under way. Others decry责难what they see as outside forces running roughshod肆意践踏over the old Harlem. New York meant Harlem to me, and as a young man I visited it whenever I could. But many of my old haunts are gone. The Theresa shut down in 1966. National chains that once ignored Harlem now anticipate yuppie money and want pieces of this prime Manhattan real estate. So here I am on a hot August afternoon, sitting in a Starbucks that two years ago opened a block away from the Theresa, snatching抓取,攫取at memories between sips of high-priced coffee. I am about to open up a piece of the old Harlem---the New York Amsterdam News---when a tourist。
文化研究:两种范式(2011年7月重译)

文化研究:两种范式(1980)斯图亚特·霍尔(Stuart Hall)孟登迎译严肃的、富有批判性的学术工作(intellectual work)没有“绝对的开端”,也鲜有不间断的连续性。
无论是思想史(History of Ideas)钟爱的对于“传统”的无限展开,还是阿尔都塞主义者(原文为Althussereans,疑为Althusserians之误——译注)偏爱的将思想(Thought)标注为“正确”或“错误”要素的“认识论断裂”的绝对论,都是如此。
相反,我们看到的是一种凌乱但带有显著特征的发展不均衡性。
重要的是那些有重大意义的断裂——陈旧的思路在此处被打断,陈旧的思想格局(constellations)被替代,而围绕一套不同的前提和主题,新旧两方面的各种因素被重新组合起来。
一个问题架构(problematic)的变化,明显转变了所提问题的本质、提问题的方式和问题可能获得充分回答的方式。
理论视角上的这些转变,不但反映出内在的学术劳动所产生的结果,而且反映出真实的历史发展和变化被纳入思想的方式及其为思想提供的存在条件——并不确保思想的“正确”,而为思想提供最根本的倾向。
正是由于思想与反映在社会思想范畴当中的历史现实之间的这种复杂的接合(articulation)以及“权力”与“知识”之间持续的辩证法(continuous dialectic),才使得这些断裂具有了记载价值。
文化研究作为一种独特的问题架构,兴起于20世纪50年代中叶那一时期。
当然,与文化研究相关的一些具体的问题,已经不是第一次被提上桌面了。
事实正好相反。
两本有助于考察这一新领域的著作——霍加特(Hoggart)的《文化素养的用途》(Uses of Literacy)和雷蒙·威廉斯的《文化与社会》——都是以不同方式(在某种程度上)重新探讨这些问题的成果。
霍加特的书参考了“文化论战”的内容,始终坚持那些有关“大众社会”的论断以及那种认同利维斯(Leavis)和《细读》(Scrutiny)的研究传统。
Unitarily invariant norms related to factors

Don Hadwin
Keywords: semi-finite factors, unitarily invariant norms, s-numbers, Ky Fan norms. MSC2000: 46L10; 46L51
1
Introduction
F.J. Murray and J.von Neumann [15, 16, 17, 26, 27] introduced and studied certain algebras of Hilbert space operators. Those algebras are now called “Von Neumann algebras.” They are strong-operator closed self-adjoint subalgebras of all bounded linear transformations on a Hilbert space. Factors are von Neumann algebras whose centers consist of scalar multiples of the identity operator. Every von Neumann algebra is a direct sum (or “direct integral”) of factors. Thus factors are the building blocks for general von Neumann algebras. Murray and von Neumann [15] classified factors into type In , I∞ , II1 , II∞ , III factors. Type In and I∞ factors are full matrix algebras: Mn (C) and B(H). Type In and II1 factors are called finite factors. There is a unique faithful normal tracial state on a finite factor. 1
Motivic integration over Deligne-Mumford stacks

a rX iv:mat h /312115v5[mat h.AG ]16Dec24MOTIVIC INTEGRATION OVER DELIGNE-MUMFORDSTACKS TAKEHIKO YASUDA Abstract.The aim of this article is to develop the theory of motivic integration over Deligne-Mumford stacks and to apply it to the birational geometry of Deligne-Mumford stacks.Contents 1.Introduction 21.1.Notation and convention 71.2.Acknowledgments 82.Stacks of twisted jets 82.1.Short review of the Deligne-Mumford stacks 82.2.Stacks of twisted jets 102.3.Morphism of stacks of twisted jets 173.Motivic integration 193.1.Convergent stacks 193.2.Convergent spaces and coarse moduli spaces 223.3.Cohomology realization 233.4.Cylinders and motivic measure 273.5.Integrals of measurable functions 283.6.Motivic integration over singular varieties 343.7.Tame proper birational morphisms and twisted arcs 373.8.Fractional Tate objects 383.9.Shift number403.10.Transformation rule414.Birational geometry of Deligne-Mumford stacks474.1.Divisors and invariants of pairs474.2.Homological McKay correspondence and discrepancies494.3.Orbifold cohomology524.4.Convergence and normal crossing divisors 542TAKEHIKO YASUDA4.5.Generalization to singular stacks57 4.6.Invariants for varieties58 References591.IntroductionIn this article,we study the motivic integration over Deligne-Mumford stacks,which was started in[Yas1].The motivic integration was intro-duced by Kontsevich[Kon]and developed by Denef and Loeser[DL1], [DL2]etc.It is now well-known that the motivic integration is effective in the study of birational geometry.For example,Batyrev[Bat]has applied it to the study of stringy E-functions and Mustat¸ˇa[Mus]to one of the singularities appearing in the minimal model program.Wefirst recall the motivic integration over varieties.Thanks to Se-bag[Seb],we can work over an arbitrary perfectfield k.Let X be a variety over k,that is,a separated algebraic space offinite type over k.For a non-negative integer n,an n-jet of X over a k-algebra R is a R[[t]]/t n+1-point of X.For each n,there exists an algebraic space J n X parameterizing n-jets.For example,J0X is X itself and J1X is the tangent bundle of X.The spaces J n X,n∈Z≥0constitute a projectivesystem and the limit J∞X:=lim←−J n X exists.We can define a measureµX and construct an integration theory on J∞X with values in some ring(or semiring)in which we can add and multiply the classes{V} of varieties V and some class of infinite sums are defined.For exam-ple,we can use a completion of the Grothendieck ring of mixed Hodge structures(k=C)or mixed Galois representations(k afinitefield).If X is smooth,then we haveJ∞X1dµX=µX(J∞X)={X}.To generalize the theory to Deligne-Mumford stacks,it is not suf-ficient to consider only R[[t]]/t n+1-points of a stack.Inspired by a work of Abramovich and Vistoli[AV],the author introduced the no-tion of twisted jets in[Yas1].Let X be a separated Deligne-Mumford stack offinite type over k andµl,k be the group scheme of l-th roots of unity for a positive integer l prime to the characteristic of k.A twisted n-jet over X is a representable morphism from a quotient stack [(Spec R[[t]]/t nl+1)/µl,k]to X.We will prove that the category J n X of twisted n-jets is a Deligne-Mumford stack.If k is algebraically closedMOTIVIC INTEGRATION OVER DELIGNE-MUMFORD STACKS3 and X is a quotient stack[M/G],then we haveJ0X∼= g∈Conj(G)[M g/C g].Here Conj(G)is a representative set of conjugacy classes,M g thefixed point locus of g and C g is the centralizer of g.The right hand side often appears in the study of McKay correspondence.There exists also the projective limit J∞X:=lim←−J n X.When X is smooth,we define a measureµX and construct an integration theory on the point set|J∞X|.Let L be the class{A1k}of an affine line.To a variety X and an ideal sheaf I⊂O X,we can associate a function ord I:J∞X→Z≥0∪{∞} and a function L ord I.Consider a proper birational morphism f:Y→X of varieties with Y smooth.The Jacobian ideal sheaf Jac f⊂O Y is defined to be the0-th Fitting ideal ofΩY/X.If X is also smooth,then this is identical with the ideal sheaf of the relative canonical divisor K Y/X:=K Y−f∗K X.Let f∞:J∞Y→J∞X be the morphism induced by f.The relation of the measuresµX andµY is described by the following transformation rule:F dµX= (F◦f∞)L−ord Jac f dµY.This formula was proved by Kontsevich[Kon],Denef and Loeser[DL1], and Sebag[Seb].Using this,we obtain many results in the birational geometry.For instance,Kontsevich proved the following:If f:Y→X and f′:Y→X′are proper birational morphisms of smooth proper varieties over C,and if K Y/X=K Y/X′,then the Hodge structure of H i(X,Q)and that of H i(X′,Q)are isomorphic.We generalize the transformation rule to Deligne-Mumford stacks. If we consider only representable morphisms,no interesting phenom-enon appears.A morphism of Deligne-Mumford stacks is said to be birational if it induces an isomorphism of open dense substacks.For example,if M is a variety with an effective action of afinite group G,then the natural morphism from the quotient stack[M/G]to the quotient variety M/G is birational.A morphism f:Y→X is said to be tame if for every geometric point y of Y,Ker(Aut(y)→Aut(f(x))) is of order prime to the characteristic of k.The transformation rule is generalized to tame,proper and birational morphisms.Let˜x be a geometric point of J0X and x its image in X.Aµl-action on the tangent space T x X derives from˜x.If for suitable basis,ζ∈µl4TAKEHIKO YASUDA acts by diag(ζa1,...,ζa d),1≤a i≤l,then we define1sht(˜x):=d−MOTIVIC INTEGRATION OVER DELIGNE-MUMFORD STACKS5 V,sht(V)∈Q is well-defined.If D=0and W=|X|,then the invariant is equal to V⊂J0X{V}L sht(V).If k=C and X is proper,this has the information of the Hodge structure of the orbifold cohomology defined below.In characteristic zero,we can generalize the invariantΣW(X,D)to the case where X is singular:A log Deligne-Mumford stack is defined to be the pair(X,D)of a normal Deligne-Mumford stack X offinite type and a Q-divisor D on X such that K X+D is Q-Cartier.For a log Deligne-Mumford stack(X,D)and a constructible subset W⊂|X|,if f:Y→X is a proper birational morphism with Y smooth,then we defineΣW(X,D):=Σf−1(W)(Y,f∗(K X+D)−K Y).This invariant is a common generalization and refinement of the stringy E-function and the orbifold E-function.By a calculation,we will see thatΣW(X,D)=∞if and only if(X,D)is Kawamata log terminal around W(For the definition,see Definition4.17).The following is the direct consequence of the transformation rule and viewed as a generalization of Batyrev’s result and Denef and Loeser’s one.Theorem1.3.Let(X,D)and(X′,D′)be log Deligne-Mumford stacks. Assume that there exist a smooth DM stack Y and proper birational morphisms f:Y→X and f′:Y→X such that f∗(K X+D)= (f′)∗(K X′+D′)and f−1(W)=(f′)−1(W′).In positive characteristic, assume in addition that X and X′are smooth and that f and f′are tame.Then we haveΣW(X,D)=ΣW′(X′,D′).Remark1.4.Kawamata[Kaw]obtained a closely related result in terms of the derived category.Finally we give corollaries of this theorem.Let G⊂GL d(C)be afinite subgroup and X:=C d/G the quotient variety.For g∈G,we define a rational number age(g)as follows: Let l be the order of g andζ:=exp(2π√ld i=1a i.If g∈SL d(C),then age(g)is an integer.The following was called the Homological McKay correspondence.It was proved by Y.Ito and Reid6TAKEHIKO YASUDA[IR]for dimension three and by Batyrev for arbitrary dimension[Bat]. (See also[Rei2]).Corollary1.5.Suppose that G⊂SL d(C)and that there is a crepant resolution Y→X.For an even integer i,putn i:=♯{g∈Conj(G)|age(g)=i/2}.Then we haveH i(Y,Q)∼= 0(i:odd)Q(−i/2)⊕n i(i:even).Since X=C d/G has only quotient singularities,K X is Q-Cartier and its pull-back by arbitrary morphism is defined.For a resolution f:Y→X and for each exceptional prime divisor E⊂Y,there is a rational number a(E,X)such thatK Y≡f∗K X+ E⊂Y a(E,X)E.The discrepancy of X is defined to be the infimum of a(E,X)for all resolutions Y→X and all exceptional divisors E⊂Y.The following is a refinement of Reid–Shepherd-Barron–Tai criterion for canonical(or terminal)quotient singularities(see[Rei1,§4.11]).Corollary1.6.For afinite group G⊂GL d(C)without reflection,the discrepancy of X=C d/G is equal tomin{age(g)|1=g∈G}−1.Chen and Ruan[CR]defined a new cohomology for topological orb-ifolds(Satake’s V-manifolds),called orbifold cohomology.We give its algebraic version.Let X be a smooth Deligne-Mumford stack over C. For i∈Q,we defineH i orb(X,Q):= V⊂J0X H i−2sht(V)(¯V,Q)⊗Q(−sht(V)).Here¯V is the coarse moduli space of V.If X is proper,then H i orb(X,Q) is a pure Hodge structure of weight i.(We define Hodge structure with fractional weights in the trivial fashion.)The following was conjectured by Ruan[Rua]and a weak version was proved by Lupercio-Poddar [LP]and the author[Yas1]independently.This is a generalization of Kontsevich’s theorem stated above.Corollary1.7.Let X and X′be proper and smooth Deligne-Mumford stacks offinite type over C.Suppose that there exist a smooth Deligne-Mumford stack Y and proper birational morphisms f:Y→X andMOTIVIC INTEGRATION OVER DELIGNE-MUMFORD STACKS7 f′:Y→X′such that K Y/X=K Y/X′.Then for every i∈Q,there is an isomorphism of Hodge structuresH i orb(X,Q)∼=H i orb(X′,Q).We also define the p-adic orbifold cohomology.Let X be a smooth Deligne-Mumford stack over afinitefield k.and p a prime number different from the characteristic of k.If necessary,replacing k with its finite extension,we defineH i orb(X⊗¯k,Q p):= V⊂J0X H i−2sht(V)(¯V⊗¯k,Q p)⊗Q p(−sht(V)).Replacing k is necessary to ensure that fractional Tate twists Q p(−sht(V)) exist.Corollary1.8.Let X and X′be proper and smooth Deligne-Mumford stacks offinite type over afinitefield k.Suppose that there exist a smooth Deligne-Mumford stack Y and tame proper birational mor-phisms f:Y→X and f′:Y→X′such that K Y/X=K Y/X′.Suppose that the p-adic orbifold cohomology groups of X and X′are defined. Then for every i∈Q,there is an isomorphism of Galois representa-tionsH i orb(X⊗k¯k,Q p)ss∼=H i orb(X′⊗k¯k,Q p)ss.Here the superscript“ss”means the semisimplification.For varieties,T.Ito[Ito1]and Wang[Wan]obtained a similar result over numberfields.1.1.Notation and convention.Throughout this paper,we work over a perfect basefield k.A Deligne-Mumford stack(DM stack for short)is supposed to be separated.What we mean by a variety is a separated algebraic space offinite type over k.•N,Z≥0:the set of positive integers and that of non-negativeintegers•[M/G]:quotient stack•|X|:the set of points of X•¯X:the coarse moduli space of a DM stack X•D l n,S:=[D nl,S/µl,k]•D n,S:=Spec R[[t]]/t n+1(S=Spec R)•µl⊂¯k:the group of l-th roots of unity•µl,k:=Spec k[x]/(x l−1):the group scheme of l-th roots ofunity over k•Conj(G):a representative set of conjugacy classes[g]of g∈G8TAKEHIKO YASUDA•Conj(µl,G):a representative set of conjugacy classes ofµl֒→G•J n X:n-jet space•J(a)n X:For a scheme with G-action and a:µl֒→G,J(a)n X⊂J n X is the locus where the twoµl-actions on J n X coincide•J l n X:the stack of twisted n-jets of order l•J n X:= char(k)∤l J l n X:the stack of twisted n-jets•πn:J∞X→J n X,π:J∞X→X:natural projections•f n:J n Y→J n X:the morphism induced by f:Y→X•R,S:the semirings of equivalence classes of convergent stacksand convergent spaces•L:={A1k}•MHS and MHS1/r:the category of mixed Hodge structuresand the category of1MOTIVIC INTEGRATION OVER DELIGNE-MUMFORD STACKS9 2.1.1.Wefirst review the Deligne-Mumford(DM)stack very briefly.We mention the book of Laumon and Moret-Bailly[LMB]as a reference of stacks.We will sometimes use results from it.Fix a basefield k.Let(Aff/k)be the category of affine schemes overk.A DM stack X is a category equipped with a functor X→(Aff/k)which satisfies several conditions.It should be afibered category over(Aff/k)and is usually best understood in terms of thefiber categories X(S),for S∈(Aff/k),and the pull-back functors f∗:X(T)→X(S) for f:S→T.The X(S)are groupoids with,at least for S offinitetype,finite automorphism groups.The DM stacks constitute a2-category.In terms of thefiber cat-egories,a1-morphism(or simply morphism)f:Y→X is the data of functors f S:Y(S)→X(S),compatible with pull-backs,and a 2-morphism f→g is a system of morphisms of functors f S→g S, compatible with pull-backs.A scheme,or more generally an algebraic space X is identified with the DM stack withfibers the discrete cat-egories with sets of objects the X(S):=Hom(S,X).A diagram of stacksX fZis said to be commutative if a2-isomorphism g◦f∼=h has been given. The strict identity g◦f=h is not required.A morphism f:Y→X of DM stacks is called representable if for every morphism M→X with M an algebraic space,thefiber product M×X Y is also an algebraic space.It is equivalent to that for every objectξ∈Y,the natural map Aut(ξ)→Aut(f(ξ))is injective.We can generalize many properties of a morphism of schemes to DM stack;´e tale,smooth,proper etc.By a condition in the definition,for every DM stack X,there exist an algebraic space M and an´e tale surjective morphism M→X,which is called an atlas.We say that X is smooth, normal etc if an atlas is so.The diagonal morphism∆:X→X×X of a DM stack X is,bydefinition,representable.We say that X is separated if∆isfinite,thatis,quasi-finite and proper.Note that∆is not immersion unless X isan algebraic space.In this paper,every DM stack is supposed to beseparated.2.1.2.Points and coarse moduli space.A point of a DM stack X isan equivalence class of morphisms Spec K→X with K⊃k afield by the following equivalence relation;morphisms Spec K1→X and10TAKEHIKO YASUDASpec K2→X are equivalent if there is anotherfield K3⊃K1,K2⊃k making the following diagram commutative.Spec K3Spec K2XWe denote by the set of the points by|X|.It carries a Zariski topology; A⊂|X|is an open subset if A=|Y|for some open immersion Y֒→X. (see[LMB]for details).If X is a scheme,then|X|is equal to the underlying topological space as sets.A coarse moduli space of a DM stack X is an algebraic space equipped with a morphism X→X such that every morphism X→Y with Y algebraic space uniquely factors through X and for every algebraically closedfield K⊃k,the map X(K)/isom→X(K)is bijective.By the definition,it is clear that the coarse moduli space is unique up to isomorphism.Keel and Mori[KM]proved that for every DM stack, the coarse moduli space exists.If X is the coarse moduli space of X, then the map|X|→|X|is a homeomorphism.2.1.3.Quotient stack.One of the simplest examples is the quotient stack.Let M be an algebraic space and G afinite group(or an´e tale finite group scheme over k)acting on M.Then we can define the quotient stack[M/G]as follows;an object over a scheme S is a pair of a G-torsor P→S and a G-equivariant morphism P→M and a morphism of(P→S,P→M)to(Q→T,Q→M)over a morphism S→T is a G-equivariant morphism P→Q compatible with the other morphisms.This stack has the canonical atlas M→[M/G].There is also a natural morphism[M/G]→M/G which makes M/G the coarse moduli space.The composition M→[M/G]→M/G is the quotient map.2.2.Stacks of twisted jets.2.2.1.In the article[Yas1],the author introduced the notion of twisted jets.There,only twisted jets overfields were considered and the stack of twisted jets was constructed as a closed substack of another stack. By contrast,in this paper,we consider the category of twisted jets parameterized by arbitrary affine scheme and verify that it is actually a DM stack.Wefirst recall jets and arcs over a variety.Here we mean a separated algebraic space offinite type by a variety.Let X be a variety and n aMOTIVIC INTEGRATION OVER DELIGNE-MUMFORD STACKS11 non-negative integer.The functor(Aff/k)→(Sets)Spec R→Hom(Spec R[[t]]/t n+1,X)is representable by a variety J n X,called the n-jet space.The nat-ural surjection k[[t]]/t n+2։k[[t]]/t n+1induces a natural projection J n+1X→J n X.Since they are all affine morphisms,the projectivelimit J∞X:=lim←−J n X exists.This is an algebraic space,but not gen-erally offinite type.We call this the arc space.For everyfield extension K⊃k,there is an identificationHom(Spec K,J∞X)=Hom(Spec K[[t]],X).An arc of X is a point of J∞X,that is,a morphism Spec K[[t]]→X. For S=Spec R∈(Aff/k)and a non-negative integer n,we putD n,S:=Spec R[[t]]/t n+1.For l a positive integer prime to the characteristic of k,we denote by µl⊂¯k the cyclic group of l-th roots of unity.We define also the group scheme of l-th roots of unity over kµl,k:=Spec k[x]/(x l−1).Whenµl,k is a constant group scheme,then we identify it with the groupµl.The natural action ofµl,k on D n,S is defined by t→x⊗t. We putD l n,S:=[D nl,S/µl,k].Also for n=∞,and for afield K⊃k,we putD∞,K:=Spec K[[t]]and D l∞,K:=[D∞,K/µl,k].Definition2.1.Let X be a DM stack.A twisted n-jet of order l of X over S is a representable morphism D l n,S→X.For afield K⊃k,a twisted arc(or twisted infinite jet)of order l of X over K is a representable morphism D l∞,K→X.Definition2.2.Let X be a DM stack.Suppose n<∞.We define the stack of twisted n-jets of order l,denoted J l n X,as follows;an object over S∈(Aff/k)is a representable morphism D l n,S→X,a morphism fromγ:D l n,S→X toγ′:D l n,T→X over f:S→T is a2-morphism fromγto f′◦γ′,where f′:D l n,S→D l n,T is the morphism naturally induced by f.We will prove that it is actually a DM stack.12TAKEHIKO YASUDADefinition 2.3.We define the stack of twisted n -jet of X byJ n X := char (k )∤lJ l n X .If X is of finite type,then J l n X is empty for sufficiently large l and J n X is in fact the disjoint sum of only finitely many J l n X .Lemma 2.4.The category J l n X is a stack.Proof.For an object γ:D l n,S →X of J l n X and for a morphism f :T →S ,we have a“pull-back”,γT :=f ′◦γwhich is unique up to 2-isomorphisms.Here f ′:D l n,T →D l n,S is the natural morphism inducedby f .Hence J l n X is a groupoid.We first show that for two objects γ,γ′:D l n,S →X ,the functorI som (γ,γ′):(Aff/S )→(Sets)(T →S )→Hom (J l n X )(T )(γT ,γ′T ).is a sheaf.Consider a morphism T →S and an ´e tale coverT i →T .Let T ij :=T i ×T T j .For every object αof D l n,T ,we have the pull-backsαi and αij to D l n,T i and D l n,T ij respectively.Since X is a prestack,the sequence0→Hom X (T )(γT (α),γ′T (α))→ Hom X (T i )(γT i (αi ),γ′T i (αi ))⇉ Hom X (T ij )(γT ij (αij ),γ′T ij(αij ))is exact.Since a morphism of twisted jets is a natural transformation of functors,it implies that the sequence0→Hom (J l n X )(T )(γT ,γ′T )→ Hom (J l n X )(T i )(γT i ,γ′T i )⇉ Hom (J l n X )(T ij )(γT ij ,γ′T ij )is also exact,and the functor I som (γ,γ′)is a sheaf.It remains to show that one can glue objects.Let T i →T be an ´e tale cover,let γi :D l n,T i →X be twisted jets and let h ij :(γi )T ij →(γj )T ij be a morphism in (J l n X )(T ij ).Assume that they satisfy thecocycle condition.Then for every object αof D l n,T ,we can glue theobjects γi (αi )of X ,because X is a stack.Therefore we can determine the image of αand obtain a functor γ:D l n,T →X which is clearly representable.Thus we have verified all conditions.MOTIVIC INTEGRATION OVER DELIGNE-MUMFORD STACKS13 2.2.2.Let Y→X be a representable morphism of DM stacks.Then for a twisted jet D l n,S→Y,composing the morphisms,we obtain atwisted jet D l n,S→X.Thus we have a natural morphism J l n Y→J l n X. In[Yas1],we defined a barely faithful morphism to be a morphism f:Y→X of DM stacks such that for every objectξof Y,the map Aut(ξ)→Aut(f(ξ))is bijective.Thus all barely faithful morphisms are representable.Barely faithful morphisms are stable under base change[Yas1,Lemma4.21].Lemma2.5.Let Y→X be a barely faithful and formally´e tale mor-phism of DM stacks.Then the naturally induced diagramJ l n Y J l n XXis cartesian.Proof.Consider a commutative diagramS YXwhere the bottom arrow is representable and the left arrow is a natural one.Then we claim that there exists a unique morphism D l n,S→Y whichfits into the diagram.The lemma easily follows from it. Without loss of generality,we can assume that S is connected.Let U⊂D l n,S×X Y be the connected component containing the image of S.Then the natural morphism U→D l n,S is barely faithful,formally ´e tale and bijective,hence an isomorphism.It shows our claim.Thus we obtain an equivalence of categories,Y×X J l n X∼=J l n Y. For every DM stack X,there arefinite groups G i,schemes M i with G i-action and a morphism i[M i/G i]→X which is´e tale,surjective and barely faithful.Hence thanks to Lemma2.5,in proving that J l n X is a DM stack,we may assume that X is a quotient stack[M/G].Let k′/k be thefield extension by adding all l-th roots of unity for the order l of elements of G prime to the characteristic of k.Replacing k with k′and M with M⊗k k′,we may assume thatµl,k is a constant group scheme for l such that there is a twisted jet D l n,S→[M/G].The action µl D n,S induces an actionµl J n M.On the other hand,for each embedding a:µl֒→G,µl acts on M as a subgroup of G and on J n M.14TAKEHIKO YASUDADefinition 2.6.We define J (a )n M to be the closed subscheme of J n Mwhere the two actions µl J n M are identical.Definition 2.7.We define Conj(µl ,G )to be a representative set of the conjugacy classes of embeddings µl ֒→G .Proposition 2.8.For 0≤n ≤∞,there is an isomorphismJ l n X ∼=a ∈Conj(µl ,G )[J (a )nl M/C a ].Here C a is the centralizer of a .By this isomorphism,[J (a )nl M/C a ]cor-responds to twisted jets D l n,S →X inducing a :µl ֒→G .Proof.Let m :=nl .Choose a primitive l -th root ζ∈µl of unity.Let γ:D l n,S →X be an object over S of J l n X .The canonical atlas D m,S →D l n,S corresponds to the object αof D l n,SD m,S ×µl µl-action D m,S D m,S.The morphismθ:=ζ×ζ−1:D m,S ×µl →D m,S ×µlis an automorphism of αover ζ:D m,S →D m,S ,whose order is l .Any other object of D l n,S is a pull-back of αand any automorphism is a pull-back of a power of θ.Therefore the twisted jet γis determined by the images of αand θin X .Let the diagramPh MD m,Sbe the object over D m,S of X which is the image of αby γ.Let λbe its automorphism over ζ:D m,S →D m,S which is the image of θ.Because γis representable,the order of λis also l .Let Q :=P ×D m,S S .Then P →D m,S is isomorphic as torsors to D m,k ×k Q →D m,k ×k S .Since we have chosen a primitive l -th root ζ,we can identify Conj(µl ,G )with a representative set Conj l (G )of the conjugacy classes of elements of order l .MOTIVIC INTEGRATION OVER DELIGNE-MUMFORD STACKS15 Claim:If S is connected,then there are open and closed subsets Q′⊂Q and P′⊂P which,for some g∈Conj l(G),are stable under C g-action and C g-torsors over S.Take an´e tale cover T→S such that Q T:=Q×S T is isomorphic to the trivial G-torsor T×G→T with a right action.Then the pull-back of the automorphismλis a left action of some g−1∈G over each connected component of T.If necessary,replacing the isomorphism Q T∼=T×G,we can assume that the automorphism is given by unique g−1∈Conj l(G).Letφ:T×G→Q be the natural morphism.Then we see thatφ(T×C g)∩φ(T×(G\C g))=∅,as follows:Let a∈C g, b∈G\C g,x∈T×C g and y∈T×(G\C g).Ifφ(x)=φ(y),then φ(x)=φ(gxg−1)=λφ(x)g−1=λφ(y)g−1=φ(gyg−1)=φ(y).It is a contradiction.Similarly P decomposes also.Since(h◦λ)|P′=h|P′and(h◦g)|P′=(g◦h)|P′,we haveh◦(ζ×id Q′)=h◦(λ◦g)|P′=(g◦h)|P′.It means that the morphism D m,k×k Q′→M corresponds to a mor-phism Q′→(J m M)ζ◦g−1and that the morphism Q′→(J m M)ζ◦g−1 and a C g-torsor Q′→S determine an object over S of a quotient stack [(J m M)ζ◦g−1/C g].Note that(J m M)ζ◦g−1=J(a)m M.Thus we have a morphism J l n X→ [J(a)m M/C a].The inverse morphism can be con-structed by following the argument conversely. Theorem2.9.Let X be a DM stack.(1)For n∈Z≥0,J l n X and J n X are DM stacks.(2)If X is offinite type(resp.smooth),then for n∈Z≥0,thenJ l n X and J n X are also offinite type(resp.smooth). (3)For every m≥n,the natural projection J m X→J n X is anaffine morphism.Proof.1:There is an´e tale,surjective and barely faithful morphism i[M/G i]→X such that each M i is a scheme and G i is afinite group.From Lemma2.5,Proposition2.8and[LMB,Lemme4.3.3], the morphism J l n X→X is representable.From[LMB,Proposition 4.5],J l n X is a DM stack.J n X is also a DM stack.The morphism J l n X→X is also separated and so is J l n X.2and3:These also result from Lemma2.5and Proposition2.8. In general,a projective system{X i,ρi:X i+1→X i}i≥0of DM stacks such that everyρi is representable and affine,there exists a projective limit X∞=lim←−X i.In fact,for each i,there is an O X0-algebra A i such that X i∼=Spec A i(see[LMB,§14.2])and the A i’s constitute an16TAKEHIKO YASUDAinductive system.We can see that X∞:=Spec(lim−→A i)is the projective limit of the given projective system.From Theorem2.9,the projective system{J n X}n(resp.{J l n X}n) has the projective limitJ∞X:=lim←−J n X(resp.J l∞X:=lim←−J l n X).Then the point set|J∞X|is identified with the set of the equivalence classes of the twisted arcs D l∞,K→X with respect to the following equivalent relation:Letγi:D l∞,Ki→X,i=1,2,be twisted arcs.If for afield K3⊃K1,K2and natural morphisms D l∞,K3→D l∞,K1,D l∞,K2,the diagramD l∞,K3D l∞,K1γ1Xis commutative,thenγ1andγ2are equivalent.Remark 2.10.For two stacks X and Y,we can define a Hom-stack H om(X,Y)which parameterizes morphisms from X to Y,and its substack H om rep(X,Y)which parameterizes representable morphisms. Olsson[Ols]proved that if X and Y are Deligne-Mumford stacks satis-fying certain conditions,then H om(X,Y)is a Deligne-Mumford stack. and H om rep(X,Y)is its open substack.Then,Aoki[Aok]proved that H om(X,Y)is an Artin stack if X and Y are Artin stacks satisfying certain conditions.The stack J l n X of twisted n-jets of order l(n<∞) is identical with H om rep(D l n,X).2.2.3.Inertia stack.Definition2.11.To each DM stack X,we associate the inertia stack I X defined as follows;an object of I X is a pair(x,α)with x an object of X andα∈Aut(x)and a morphism(x,α)→(y,β)in I X is a morphismφ:x→y in X withφα=βφ.It is known that I X is isomorphic to X×∆,X×X,∆X,where∆: X→X×X is the diagonal morphism.Then the forgetting morphism I X→X is isomorphic to thefirst projection X×∆,X×X,∆X→X. Since we have supposed that X is separated,the diagonal morphism is finite and unramified.Hence the forgetting morphism I X→X is so as well.Definition2.12.Let l be a positive integer prime to char(k).We define I l X⊂I X to be the open and closed substack of objects(x,α) such that the order ofαis l.MOTIVIC INTEGRATION OVER DELIGNE-MUMFORD STACKS17 Proposition 2.13.Assume that k contains all l-th roots of unity. Then for each choice of a primitive l-th rootζof unity,there is anatural isomorphism J l0X∼=I l X.Proof.The assertion follows from the fact that giving a representable morphism D l0×S→X is equivalent to giving an object x over S of X and an embeddingµl֒→Aut(x),which is equivalent to giving the image ofζ∈µl. The inertia stack is the algebraic counterpart of the twisted sector ofan analytic orbifold,which was used to define the orbifold cohomology√in[CR].Since for k=C,there is a canonical choice exp(2π。
KMS states and complex multiplication

a r X i v :m a t h /0501424v 1 [m a t h .O A ] 24 J a n 2005KMS STATES AND COMPLEX MULTIPLICATIONALAIN CONNES,MATILDE MARCOLLI,AND NIRANJAN RAMACHANDRAN1.Introduction Several results point to deep relations between noncommutative geometry and class field theory ([2],[9],[18],[20]).In [2]a quantum statistical mechanical system is exhibited,with partition function the Riemann zeta function,and whose arithmetic properties are related to the Galois theory of the maximal abelian extension of Q .In [9],this system is reinterpreted in terms of the geometry of commensurable 1-dimensional Q -lattices,and a generalization is constructed for 2-dimensional Q -lattices.The arithmetic properties of this GL 2-system and its KMS states at zero temperature related to the Galois theory of the modular field.The ground states and the Galois properties are analyzed in [9]for the generic case of elliptic curves with transcendental j -invariant.As the results of [9]show,one of the main new features of the GL 2-system is the presence of symmetries by endomorphism ,through which the full Galois group of the modular field appears as symmetries acting on the KMS equilibrium states of the system.In both the original BC system and in the GL 2-system,the arithmetic properties of zero temperature KMS states rely on an underlying result of compatibility between ad`e lic groups of symmetries and Galois groups.This correspondence between ad`e lic and Galois groups naturally arises within the context of Shimura varieties.In fact,a Shimura variety is a pro-variety defined over Q ,with a rich ad`e lic group of symmetries.In that context,the compatibility of the Galois action and the automorphisms is at the heart of Langlands program.This leads us to give a reinterpretation of the BC and the GL 2systems in the language of Shimura varieties,with the BC system corresponding to the simplest (zero dimensional)Shimura variety.In the case of the GL 2system,we show how the data of 2-dimensional Q -lattices and commensurability can be also described in terms of elliptic curves together with a pair of points in the total Tate module,and the system is related to the Shimura variety of GL2.This viewpoint suggests considering our systems as noncommutative pro-varieties defined over Q ,more specifically as noncommutative Shimura varieties.We then present our main result,which is the construction of a new system,whose arithmetic prop-erties fully incorporate the explicit class field theory for an imaginary quadratic field K ,and whose partition function is the Dedekind zeta function of K .The underlying geometric structure is given by commensurability of 1-dimensional K -lattices.This new system can be regarded in two different ways.On the one hand,it is a generalization ofthe BC system of [2],when changing the field from Q to K ,and is in fact Morita equivalent to the one considered in [18],but with no restriction on the class number.On the other hand,it is also a specialization of the GL 2-system of [9]to elliptic curves with complex multiplication by K .In this case the ground states can be related to the non-generic ground states of the GL 2-system,associated to points τ∈H with complex multiplication,and the group of symmetries is the Galois group of the maximal abelian extension of K .Here also we show that symmetries by endomorphisms play a crucial role,as they allow for the action of the class group Cl(O ),so that our results hold for any class field.Since this complex multiplication (CM)case can be realized as a subgroupoid of the GL 2-system,it has a natural choice of a rational subalgebra (an arithmetic structure)inherited from that of the GL 2-system.This is crucial,in order to obtain the intertwining of Galois action on the values of extremal states and action of symmetries of the system.12CONNES,MARCOLLI,AND RAMACHANDRANWe summarize and compare the main properties of the three systems(BC,GL2,and CM)in the following table.GL1CMpartition functionζ(β)ζ(β−1)A∗/Q∗A∗K,f/K∗fautomorphisms GL2(ˆZ)Cl(O)Galois group Aut(F)Sh(GL1,±1)A∗K,f/K∗The paper consists of two parts,with sections2and3centered on the relation of the BC and GL2 system to the arithmetic of Shimura varieties,and sections4and5dedicated to the construction of the CM system and its relation to the explicit classfield theory for imaginary quadraticfields.The two parts are closely interrelated,but can also be read independently.2.Quantum Statistical Mechanics and Explicit Class Field TheoryThe BC quantum statistical mechanical system[1,2]exhibits generators of the maximal abelian extension of Q,parameterizing ground states(i.e.at zero temperature).Moreover,the system has the remarkable property that these ground states take algebraic values,when evaluated on a rational subalgebra of the C∗-algebra of observables.The action on these values of the absolute Galois group factors through the abelianization Gal(Q ab/Q)and is implemented by the action of the id`e le class group as symmetries of the system,via the classfield theory isomorphism.This suggests the intriguing possibility of using the setting of quantum statistical mechanics to address the problem of explicit classfield theory for other numberfields.In this section we recall some basic notions of quantum statistical mechanics and of classfield theory, which will be used throughout the paper.We also formulate a general conjectural relation between quantum statistical mechanics and the explicit classfield theory problem for numberfields.Quantum Statistical Mechanics.A quantum statistical mechanical system consists of an algebra of observables,given by a unital C∗-algebra A,together with a time evolution,consisting of a1-parameter group of automorphismsσt, (t∈R),whose infinitesimal generator is the Hamiltonian of the system.The analog of a probability measure,assigning to every observable a certain average,is given by a state,namely a continuous linear functionalϕ:A→C satisfying positivity,ϕ(x∗x)≥0,for all x∈A,and normalization,ϕ(1)=1.In the quantum mechanical framework,the analog of the classical Gibbs measure is given by states satisfying the KMS condition(cf.[13]).Definition2.1.A triple(A,σt,ϕ)satisfies the Kubo-Martin-Schwinger(KMS)condition at inverse temperature0≤β<∞,if,for all x,y∈A,there exists a bounded holomorphic function F x,y(z)on the strip0<Im(z)<β,continuous on the boundary of the strip,such that(2.1)F x,y(t)=ϕ(xσt(y))and F x,y(t+iβ)=ϕ(σt(y)x),∀t∈R.KMS AND CM3 We also say thatϕis a KMSβstate for(A,σt).The set Kβof KMSβstates is a compact convex Choquet simplex[3,II§5]whose set of extreme points Eβconsists of the factor states.At0temperature(β=∞)the KMS condition(2.1)says that,for all x,y∈A,the function(2.2)F x,y(t)=ϕ(xσt(y))extends to a bounded holomorphic function in the upper half plane H.This implies that,in the Hilbert space of the GNS representation ofϕ(i.e.the completion of A in the inner productϕ(x∗y)), the generator H of the one-parameter groupσt is a positive operator(positive energy condition). However,this notion of0-temperature KMS states is in general too weak,hence the notion of KMS∞states that we shall consider is the following.Definition2.2.A stateϕis a KMS∞state for(A,σt)if it is a weak limit ofβ-KMS states for β→∞.One can easily see the difference between these two notions in the case of the trivial evolutionσt= id,∀t∈R,where any state has the property that(2.2)extends to the upper half plane(as a constant),while weak limits ofβ-KMS states are automatically tracial states.With Definition2.2we still obtain a weakly compact convex setΣ∞and we can consider the set E∞of its extremal points.The typical framework for spontaneous symmetry breaking in a system with a unique phase transition (cf.[12])is that the simplexΣβconsists of a single point forβ≦βc i.e.when the temperature is larger than the critical temperature T c,and is non-trivial(of some higher dimension in general)when the temperature lowers.A(compact)group of automorphisms G⊂Aut(A)commuting with the time evolution,(2.3)σtαg=αgσt∀g∈G,t∈R,is a symmetry group of the system.Such G acts onΣβfor anyβ,hence on the extreme points E(Σβ)=Eβ.The choice of an equilibrium stateϕ∈Eβmay break this symmetry to a smaller subgroup given by the isotropy group Gϕ={g∈G,gϕ=ϕ}.The unitary group U of thefixed point algebra ofσt acts by inner automorphisms of the dynamical system(A,σt),by(2.4)(Ad u)(a):=u a u∗,∀a∈A,for all u∈U.One can define an action modulo inner of a group G on the system(A,σt)as a map α:G→Aut(A,σt)fulfilling the condition(2.5)α(gh)α(h)−1α(g)−1∈Inn(A,σt),∀g,h∈G,i.e.,as a homomorphism of G to Aut(A,σt)/U.The KMSβcondition shows that the inner automor-phisms Inn(A,σt)act trivially on KMSβstates,hence(2.5)induces an action of the group G on the setΣβof KMSβstates,for0<β≤∞.More generally,one can consider actions by endomorphisms(cf.[9]),where an endomorphismρof the dynamical system(A,σt)is a∗-homomorphismρ:A→A commuting with the evolutionσt.There is an induced action ofρon KMSβstates,for0<β<∞,given by(2.6)ρ∗(ϕ):=Z−1ϕ◦ρ,Z=ϕ(e),provided thatϕ(e)=0,where e=ρ(1)is an idempotentfixed byσt.An isometry u∈A,u∗u=1,satisfyingσt(u)=λit u for all t∈R and for someλ∈R∗+,defines an inner endomorphism Ad u of the dynamical system(A,σt),again of the form(2.4).The KMSβcondition shows that the induced action of Ad u onΣβis trivial,cf.[9].The induced action(modulo inner)of a semigroup of endomorphisms of(A,σt)on the KMSβstates in general may not extend directly to KMS∞states(in a nontrivial way),but it may be defined on E∞by“warming up and4CONNES,MARCOLLI,AND RAMACHANDRANcooling down”(cf.[9]),provided the“warming up”map Wβ:E∞→Eβis a bijection between KMS∞states(in the sense of Definition2.2)and KMSβstates,for sufficiently largeβ.The map is given byTr(πϕ(a)e−βH)(2.7)Wβ(ϕ)(a)=−d),for some positive integer d>1.We denote byˆZ the profinite completion of Z and by A f=ˆZ⊗Q the ring offinite adeles of Q.For any abelian group G,we denote by G tors the subgroup of elements offinite order.For any ring R,we write R∗for the group of invertible elements,while R×denotes the set of nonzero elements of R,which is a semigroup if R is an integral domain.We write O for the ring of algebraic integers of K.We setˆO:=(O⊗ˆZ)and write A K,f=A f⊗Q K and I K=A∗K,f=GL1(A K,f). Note that K∗embeds diagonally into I K.The classfield theory isomorphism provides the canonical identification(2.8)θ:I K/K∗∼−→Gal(K ab/K),with K∗replaced by Q∗+when K=Q.KMS AND CM5Fabulous states for numberfields.The connection between classfield theory and quantum statistical mechanics can be formulated as the problem of constructing a class of quantum statistical mechanical systems,whose set of ground states E∞has special arithmetic properties,because of which we refer to such states as“fabulous states”. Given a numberfield K,with a choice of an embedding K⊂C,the“problem of fabulous states”consists of constructing a C∗-dynamical system(A,σt),with an arithmetic subalgebra A Q of A,with the following properties:(1)The quotient group G=C K/D K acts on A as symmetries compatible withσt.(2)The statesϕ∈E∞,evaluated on elements of the arithmetic subalgebra A Q,satisfy:•ϕ(a)∈6CONNES,MARCOLLI,AND RAMACHANDRANaction(by S=R∗+in the1-dimensional case,or by S=C∗in the2-dimensional case),the algebra of coordinates associated to the quotient R/S is obtained by restricting the convolution product of thealgebra of R to weight zero functions with S-compact support.The algebra obtained this way,whichis unital in the1-dimensional case,but not in the2-dimensional case,has a natural time evolution given by the ratio of the covolumes of a pair of commensurable lattices.Every unit y∈R(0)of Rdefines a representationπy by left convolution of the algebra of R on the Hilbert space H y=ℓ2(R y),where R y is the set of elements with source y.This construction passes to the quotient by the scaling action of S.Representations corresponding to points that acquire a nontrivial automorphism groupwill no longer be irreducible.If the unit y∈R(0)corresponds to an invertible Q-lattice,thenπy is apositive energy representation.In both the1-dimensional and the2-dimensional case,the set of extremal KMS states at low tem-perature is given by a classical ad`e lic quotient,namely,by the Shimura varieties for GL1and GL2,respectively,hence we argue here that the noncommutative space describing commensurability classes of Q-lattices up to scale can be thought of as a noncommutative Shimura variety,whose set of classicalpoints is the corresponding classical Shimura variety.In both cases,a crucial step for the arithmetic properties of the action of symmetries on extremal KMS states at zero temperature is the choice of an arithmetic subalgebra of the system,on whichthe ground states are evaluated.Such choice gives the underlying noncommutative space a more rigidstructure,of“noncommutative arithmetic variety”.Tower Power.If V is an algebraic variety–or a scheme or a stack–over afield k,a“tower”T over V is a family V i(i∈I)offinite(possibly branched)covers of V such that for any i,j∈I,there is a l∈I with V l a cover of V i and V j.Thus,I is a partially ordered set.In case of a tower over a pointed variety(V,v),onefixes a point v i over v in each V i.Even though V i may not be irreducible,we shall allow ourselves to loosely refer to V i as a variety.It is convenient to view a“tower”T as a category C with objects(V i→V)and morphisms Hom(V i,V j)being maps of covers of V.One has the group Aut T(V i)of invertible self-maps of V i over V(the group of deck transformations);the deck transformations are not required to preserve the points v i.There is a(profinite)group of symmetries associated to a tower,namely(3.2)G:=lim←−i Aut T(V i).The simplest example of a tower is the“fundamental group”tower associated with a(smooth con-nected)complex algebraic variety(V,v)and its universal covering(˜V,˜v).Let C be the category of all finite´e tale(unbranched)intermediate covers˜V→W→V of V.In this case,the symmetry group G of(3.2)is the algebraic fundamental group of V;it is also the profinite completion of the(topological) fundamental groupπ1(V,v).Simple variants of this example include allowing controlled ramification. Other examples of towers are those defined by iteration of self maps of algebraic varieties.For us,the most important examples of“towers”will be the cyclotomic tower and the modular tower.Another very interesting case of towers is that of more general Shimura varieties.These,however,will not be treated in this paper.(For a more general treatment of noncommutative Shimura varieties see [11].)The cyclotomic tower and the BC system.In the case of Q,an explicit description of Q ab is provided by the Kronecker–Weber theorem.Thisshows that thefield Q ab is equal to Q cyc,thefield obtained by attaching all roots of unity to Q. Namely,Q ab is obtained by attaching the values of the exponential function exp(2πiz)at the torsion points of the circle group R/ing the isomorphism of abelian groups¯Q∗tors∼=Q/Z and the identification Aut(Q/Z)=GL1(ˆZ)=ˆZ∗,the restriction to¯Q∗tors of the natural action of Gal(¯Q/Q) on¯Q∗factors asGal(¯Q/Q)→Gal(¯Q/Q)ab=Gal(Q ab/Q)∼−→ˆZ∗.KMS AND CM7 Geometrically,the above setting can be understood in terms of the cyclotomic tower.This has base Spec Z=V1.The family is Spec Z[ζn]=V n whereζn is a primitive n-th root of unity(n∈N∗). The set Hom(V m→V n),non-trivial for n|m,corresponds to the map Z[ζn]֒→Z[ζm]of rings.Thegroup Aut(V n)=GL1(Z/n Z)is the Galois group Gal(Q(ζn)/Q).The group of symmetries(3.2)of the tower is then(3.3)G=lim←−n GL1(Z/n Z)=GL1(ˆZ),which is isomorphic to the Galois group Gal(Q ab/Q)of the maximal abelian extension of Q.The classical object that we consider,associated to the cyclotomic tower,is the Shimura variety given by the ad`e lic quotient(3.4)Sh(GL1,{±1})=GL1(Q)\(GL1(A f)×{±1})=A∗f/Q∗+.Now we consider the space of1-dimensional Q-lattices up to scaling modulo commensurability.This can be described as follows([9]).In one dimension,every Q-lattice is of the form(3.5)(Λ,φ)=(λZ,λρ),for someλ>0and someρ∈Hom(Q/Z,Q/Z).Since we can identify Hom(Q/Z,Q/Z)endowed with the topology of pointwise convergence with(3.6)Hom(Q/Z,Q/Z)=lim←−n Z/n Z=ˆZ,we obtain that the algebra C(ˆZ)is the algebra of coordinates of the space of1-dimensional Q-lattices up to scaling.The groupˆZ is the Pontrjagin dual of Q/Z,hence we also have an identification C(ˆZ)=C∗(Q/Z).The group of deck transformations G=ˆZ∗of the cyclotomic tower acts by automorphisms on the algebra of coordinates C(ˆZ).In addition to this action,there is a semigroup action of N×=Z>0 implementing the commensurability relation.This is given by endomorphisms that move vertically across the levels of the cyclotomic tower.They are given by(3.7)αn(f)(ρ)=f(n−1ρ),∀ρ∈nˆZ.Namely,αn is the isomorphism of C(ˆZ)with the reduced algebra C(ˆZ)πby the projectionπn givennby the characteristic function of nˆZ⊂ˆZ.Notice that the action(3.7)cannot be restricted to the set of invertible Q-lattices,since the range ofπn is disjoint from them.The algebra of coordinates A1on the noncommutative space of equivalence classes of1-dimensional Q-lattices modulo scaling,with respect to the equivalence relation of commensurability,is given then by the semigroup crossed product(3.8)A=C(ˆZ)⋊αN×.Equivalently,we are considering the convolution algebra of the groupoid R1/R∗+given by the quotient by scaling of the groupoid of the equivalence relation of commensurability on1-dimensional Q-lattices, namely,R1/R∗+has as algebra of coordinates the functions f(r,ρ),forρ∈ˆZ and r∈Q∗such that rρ∈ˆZ,with the convolution product(3.9)f1∗f2(r,ρ)= f1(rs−1,sρ)f2(s,ρ),and the adjoint f∗(r,ρ)=8CONNES,MARCOLLI,AND RAMACHANDRANAs a set,the space of commensurability classes of1-dimensional Q-lattices up to scaling can also be described by the quotient(3.10)GL1(Q)\A·/R∗+=GL1(Q)\(A f×{±1}),where A·:=A f×R∗is the set of ad`e les with nonzero archimedean component.Rather than considering this quotient set theoretically,we regard it as a noncommutative space,so as to be able to extend to it the ordinary tools of geometry that can be applied to the“good”quotient(3.4).The noncommutative algebra of coordinates of(3.10)is the crossed product(3.11)C0(A f)⋊Q∗+.This is Morita equivalent to the algebra(3.8).In fact,(3.8)is obtained as a full corner of(3.11),C(ˆZ)⋊N×= C0(A f)⋊Q∗+ π,by compression with the projectionπgiven by the characteristic function ofˆZ⊂A f(cf.[17]).The quotient(3.10)with its noncommutative algebra of coordinates(3.11)can then be thought of as the noncommutative Shimura variety(3.12)Sh(nc)(GL1,{±1}):=GL1(Q)\(A f×{±1})=GL1(Q)\A·/R∗+,whose set of classical points is the well behaved quotient(3.4).This has a compactification,obtained by replacing A·by A,as in[7],(3.13)−1)c(Z)=1.One considers the rational subalgebra A1,Q of(3.8)generated by the functions e1,a(r,ρ):=e1,a(ρ)and by the functionsµn(r,ρ)=1for r=n and zero otherwise,that implement the semigroup action of N×in(3.8).As proved in[9],the algebra A1,Q is the same as the rational subalgebra considered in[2],generated over Q by theµn and the exponential functions(3.17)e(r)(ρ):=exp(2πiρ(r)),forρ∈Hom(Q/Z,Q/Z),and r∈Q/Z,KMS AND CM9 with relations e(r+s)=e(r)e(s),e(0)=1,e(r)∗=e(−r),µ∗nµn=1,µkµn=µkn,and(3.18)µn e(r)µ∗n=1ζ(β)∞n=1n−βρ(ζk r).•The group GL1(ˆZ)acts by automorphisms of the system.The induced action of GL1(ˆZ)on the set of extreme KMS states below critical temperature is free and transitive.•The vacuum states(β=∞)are fabulous states for thefield K=Q,namelyϕ(A1,Q)⊂Q cycl and the classfield theory isomorphismθ:Gal(Q cycl/Q)∼=→ˆZ∗intertwines the Galois action on values with the action ofˆZ∗by symmetries,(3.21)γϕ(x)=ϕ(θ(γ)x),for allϕ∈E∞,for allγ∈Gal(Q cycl/Q)and for all x∈A1,Q.The modular tower and the GL2-system.Modular curves arise as moduli spaces of elliptic curves endowed with additional level structure.Every congruence subgroupΓ′ofΓ=SL2(Z)defines a modular curve YΓ′;we denote by XΓ′the smooth compactification of the affine curve YΓ′obtained by adding cusp points.Especially important among these are the modular curves Y(n)and X(n)corresponding to the principal congruence subgroups Γ(n)for n∈N∗.Any XΓ′is dominated by an X(n).We refer to[15,29]for more details.We have the following descriptions of the modular tower.10CONNES,MARCOLLI,AND RAMACHANDRANCompact version:The base is V=P1over Q.The family is given by the modular curves X(n),consid-ered over the cyclotomicfield Q(ζn)[23].We note that GL2(Z/n Z)/±1is the group of automorphisms of the projection V n=X(n)→X(1)=V1=V.Thus,we have(3.22)G=GL2(ˆZ)/±1=lim←−n GL2(Z/n Z)/{±1}.Non-compact version:The open modular curves Y(n)form a tower with base the j-line Spec Q[j]= A1=V1−{∞}.The ring of modular functions is the union of the rings of functions of the Y(n),with coefficients in Q(ζn)[15].This shows how the modular tower is a natural geometric way of passing from GL1(ˆZ)to GL2(ˆZ). The formulation that is most convenient in our setting is the one given in terms of Shimura varieties. In fact,rather than the modular tower defined by the projective limit(3.23)Y=lim←−n Y(n)of the modular curves Y(n),it is better for our purposes to consider the Shimura variety(3.24)Sh(H±,GL2)=GL2(Q)\(GL2(A f)×H±)=GL2(Q)\GL2(A)/C∗,of which(3.23)is a connected component.In fact,it is well known that,for arithmetic purposes,it is always better to work with nonconnected rather than with connected Shimura varieties(cf.e.g.[23]). The simple reason why it is necessary to pass to the nonconnected case is the following.The varieties in the tower are arithmetic varieties defined over numberfields.However,the numberfield typically changes along the levels of the tower(Y(n)is defined over the cyclotomicfield Q(ζn)).Passing to nonconnected Shimura varieties allows precisely for the definition of a canonical model where the whole tower is defined over the same numberfield.This distinction is important to our viewpoint,since we want to work with noncommutative spaces endowed with an arithmetic structure,specified by the choice of an arithmetic subalgebra.Every2-dimensional Q-lattice can be described by data(3.25)(Λ,φ)=(λ(Z+Z z),λα),for someλ∈C∗,some z∈H,andα∈M2(ˆZ)(using the basis(1,−z)of Z+Z z as in(87)[9]to view αas a mapφ).The diagonal action ofΓ=SL2(Z)yields isomorphic Q-lattices,and(cf.(87)[9])the space of2-dimensional Q-lattice up to scaling can be identified with the quotient(3.26)Γ\(M2(ˆZ)×H).The relation of commensurability is implemented by the partially defined action of GL+2(Q)on(3.26). The groupoid R2of the commensurability relation on2-dimensional Q-lattices not up to scaling(i.e. the dual space)has as algebra of coordinates the convolution algebra ofΓ×Γ-invariant functions on (3.27)˜U={(g,α,u)∈GL+2(Q)×M2(ˆZ)×GL+2(R)|gα∈M2(ˆZ)}.Up to Morita equivalence,this can also be described as the crossed product(3.28)C0(M2(A f)×GL2(R))⋊GL2(Q).When we pass to Q-lattices up to scaling,we take the quotient R2/C∗.If(Λk,φk)k=1,2are a pair of commensurable2-dimensional Q-lattices,then for anyλ∈C∗,the Q-lattices(λΛk,λφk)are also commensurable,withr(g,α,uλ)=λ−1r(g,α,u).However,the action of C∗on Q-lattices is not free due to the presence of lattices L=(0,z),where z∈Γ\H has nontrivial automorphisms.Thus,the quotient Z=R2/C∗is no longer a groupoid.This can be seen in the following sim-ple example.Consider the two Q-lattices(α1,z1)=(0,2i)and(α2,z2)=(0,i).The composite ((α1,z1),(α2,z2))◦((α2,z2),(α1,z1))is equal to the identity((α1,z1),(α1,z1)).We can also considerthe composition (i (α1,z 1),i (α2,z 2))◦((α2,z 2),(α1,z 1)),where i (α2,z 2)=(α2,z 2),but this is not the identity,since i (α1,z 1)=(α1,z 1).However,we can still consider the convolution algebra of Z ,by restricting the convolution product of R 2to homogeneous functions of weight zero with C ∗-compact support,where a function f has weight k if it satisfiesf (g,α,uλ)=λk f (g,α,u ),∀λ∈C ∗.This is the analog of the description (3.8)for the 1-dimensional case.The noncommutative algebra of coordinates A 2is thus given by a Hecke algebra of functions on(3.29)U ={(g,α,z )∈GL +2(Q )×M 2(ˆZ )×H ,gα∈M 2(ˆZ )}invariant under the Γ×Γaction(3.30)(g,α,z )→(γ1gγ−12,γ2α,γ2(z )),with convolution(3.31)(f 1∗f 2)(g,α,z )= s ∈Γ\GL +2(Q ),sα∈M 2(ˆZ )f 1(gs −1,sα,s (z ))f 2(s,α,z )and adjoint f ∗(g,α,z )=Another reformulation uses the Pontrjagin duality between profinite abelian groups and discrete tor-sion abelian groups given by Hom(−,Q/Z).This reformulates the datumφof a Q-lattice as aˆZ-linear map Hom(QΛ/Λ,Q/Z)→ˆZ⊕ˆZ,which is identified withΛ⊗ˆZ→ˆZ⊕ˆZ.Here we use the fact that ΛandΛ⊗ˆZ∼=H1(E,ˆZ)are both self-dual(Poincar´e duality of E).In this dual formulation com-mensurability means that the two maps agree on the intersection of the two commensurable lattices,(Λ1∩Λ2)⊗ˆZ.With the formulation of Proposition3.2,can then give a new interpretation of the result of Proposition 43of[9],which shows that the space of commensurability classes of2-dimensional Q-lattices up to scaling is described by the quotient(3.36)Sh(nc)(H±,GL2):=GL2(Q)\(M2(A f)×H±).In fact,the data(Λ,φ)of a Q-lattice in C are equivalent to data(E,η)of an elliptic curve E=C/Λand an A f-homomorphism(3.37)η:Q2⊗A f→Λ⊗A f,withΛ⊗A f=(Λ⊗ˆZ)⊗Q,where we can identifyΛ⊗ˆZ with the total Tate module of E,as in(3.35). Since the Q-lattice need not be invertible,we do not require thatηbe an A f-isomorphism(cf.[23]). The commensurability relation between Q-lattices corresponds to the equivalence(E,η)∼(E′,η′) given by an isogeny g:E→E′andη′=(g⊗1)◦η.Namely,the equivalence classes can be identified with the quotient of M2(A f)×H±by the action of GL2(Q),(ρ,z)→(gρ,g(z)).Thus,(3.36)describes a noncommutative Shimura variety which has the Shimura variety(3.24)as the set of its classical points.The results of[9]show that,as in the case of the BC system,one recovers the classical points from the low temperature extremal KMS states.We shall return to this in the next section.In this case,the“compactification”,analogous to passing from(3.10)to(3.14),corresponds to the replacing(3.36)by the noncommutative space(3.38)in[31].This suggests that modular functions should appear naturally in the arithmetic subalgebra A2,Q of the GL2-system,but that requires working with unbounded multipliers.This is indeed the case for the arithmetic subalgebra A2,Q defined in[9],which we now recall.Let F be the modularfield,namely thefield of modular functions over Q ab(cf.e.g.[19]).This is the union of thefields F N of modular functions of level N rational over the cyclotomicfield Q(ζN),that is,such that the q-expansion at a cusp has coefficients in the cyclotomicfield Q(ζN).The action of the Galois groupˆZ∗≃Gal(Q ab/Q)on the coefficients of the q-expansion determines a homomorphism(3.42)cycl:ˆZ∗→Aut(F).If f is a continuous functions on Z=R2/C∗,we writef(g,α)(z)=f(g,α,z)so that f(g,α)∈C(H).For p N:M2(ˆZ)→M2(Z/N Z)the canonical projection,we say that f is of level N iff(g,α)=f(g,p(α))∀(g,α).NThen f is completely determined by the functionsf(g,m)∈C(H),for m∈M2(Z/N Z).Notice that the invariance f(gγ,α,z)=f(g,γα,γ(z)),for allγ∈Γand for all(g,α,z)∈U,implies that f(g,m)|γ=f(g,m),for allγ∈Γ(N)∩g−1Γg,i.e.f is invariant under a congruence subgroup. The arithmetic algebra A2,Q defined in[9]is a subalgebra of continuous functions on Z=R2/C∗with the convolutions product(3.31)and with the properties:•The support of f inΓ\GL+2(Q)isfinite.•The function f is offinite level withf(g,m)∈F∀(g,m).•The function f satisfies the cyclotomic condition:f(g,α(u)m)=cycl(u)f(g,m),for all g∈GL+2(Q)diagonal and all u∈ˆZ∗,withα(u)= u001and cycl as in(3.42).Here F is the modularfield,namely thefield of modular functions over Q ab.This is the union of the fields F N of modular functions of level N rational over the cyclotomicfield Q(ζN).The cycloomic condition is a consistency condition on the roots of unity that appear in the coefficients of the q-series,which allows for the existence of“fabulous states”(cf.[9]).Forα∈M2(ˆZ),let Gα⊂GL+2(Q)be the set ofGα={g∈GL+2(Q):gα∈M2(ˆZ)}.Then,as shown in[9],an element y=(α,z)∈M2(ˆZ)×H determines a unitary representation of the Hecke algebra A on the Hilbert spaceℓ2(Γ\Gα),(3.43)((πy f)ξ)(g):= s∈Γ\Gαf(gs−1,sα,s(z))ξ(s),∀g∈Gαfor f∈A andξ∈ℓ2(Γ\Gα).Invertible Q-lattices determine positive energy representations,due to the fact that the condition gα∈M2(ˆZ)for g∈GL+2(Q)andα∈GL2(ˆZ)(invertible case)implies g∈M2(Z)+,hence the time evolution(3.32)is implemented by the positive Hamiltonian with spectrum{log det(m)}⊂[0,∞)for。
爱默生简介中英文对照

---------方涵琦
生平简介
• 拉尔夫· 沃尔多· 爱默生(Ralph Waldo Emerson, 1803年-1882年),生于波士顿。 美国思想家、 文学家,诗人。 爱默生是确立美国文化精神的代 表人物。 美国前总统林肯称他为“美国的孔子”、 “美国文明之父”。1803年5月6日出生于马萨诸 塞州波士顿附近的康考德村,1882年4月27日在 波士顿逝世。他的生命几乎横贯19世纪的美国, 他出生时候的美国热闹却混沌,一些人意识到它 代表着某种新力量的崛起,却无人能够清晰的表 达出来。
Ralph Waldo Emerson (May 25, 1803 – April 27, 1882) was an American lecturer, philosopher, essayist, and poet, best remembered for leading the Transcendentalist movement of the mid-19th century. He was seen as a champion of individualism and a prescient critic of the countervailing pressures of society, and he disseminated his thoughts through dozens of published essays and more than 1,500 public lectures across the United States. Emerson gradually moved away from the religious and social beliefs of his contemporaries, formulating and expressing the philosophy of Transcendentalism in his 1836 essay, Nature. Following this ground-breaking work, he gave a speech entitled The American Scholar in 1837, which Oliver Wendell Holmes, Sr. considered to be America's "Intellectual Declaration of Independence".[1] Considered one of the great lecturers of the time, Emerson had an enthusiasm and respect for his audience that enraptured crowds.
the structural study of myth

the structural study of mythThe structural study of myth is a field of research that focuses on understanding the underlying patterns and structures that exist within myths across different cultures. This approach analyzes the various elements, themes, and narrative structures of myths to identify recurring patterns and archetypes.One of the key figures in the structural study of myth is French anthropologist Claude Lévi-Strauss, who introduced structuralism as a method of analysis. According to Lévi-Strauss, myths are not simply random stories but have deep-rooted underlying structures that reflect universal cognitive patterns.Structural analysis of myth typically involves identifying binary oppositions, such as good versus evil or order versus chaos, that are present within a myth. These oppositions help to create tension and structure the narrative. Scholars also examine the roles of characters, the relationships between them, and the symbolic meanings embedded within the myth.Through this approach, researchers aim to uncover the underlying cultural and psychological significance of myths. They argue that myths serve as a way for societies to understand and represent fundamental aspects of human experience, such as creation, death, and morality.The structural study of myth has been influential in various disciplines, including anthropology, sociology, psychology, and literary theory. It offers a framework for analyzing and interpretingmyths from a cross-cultural perspective, revealing the common structural elements that underlie human storytelling.。
