英文版 微积分试卷答案 (1)
微积分英文版第九版课后练习题含答案

微积分英文版第九版课后练习题含答案微积分是数学中重要的一部分,其关注的是一条曲线或曲面的局部性质。
美国著名数学家Stewart所著的微积分英文版第九版是微积分学习的重要教材之一,本文将介绍其课后练习题,并提供答案供大家进行自我学习和测试。
课后练习题微积分英文版第九版的课后练习题共分为两部分,其中Part 1是选择题,Part 2是填空题和证明题,共计约1700道题目。
Part 1中包含了大量的选择题,这些题目主要考察读者对微积分理论的掌握和应用。
大多数题目都要求读者用所学知识推理或计算来获得正确答案。
这些题目中难易程度参差不齐,有一些比较简单,但也有一些比较困难。
Part 2的题目类型较多,包括了填空题、证明题、计算题等。
这些题目内容繁杂、难度较大,需要读者花费很多时间和精力来解答。
这些题目主要是为了检测读者对所学知识的深层次理解和应用能力,考察读者的逻辑思维和推理能力。
答案微积分英文版第九版提供了相应的课后练习答案,可以帮助读者检验自己的答案是否正确,同时也可以帮助读者更好地理解和掌握所学知识。
答案分为Part 1和Part 2两部分,且每部分分别包含了选择题和非选择题的答案。
这些答案详细、准确,提供了完整的解题思路和方法,帮助读者更好地理解题目的解法,并弥补了部分教材中的不足之处。
读者可以通过该教材的官方网站或者其他渠道获得课后练习题的答案。
结论微积分是数学中非常重要的一门学科,对于各个领域的科学研究、技术发展和社会进步都有着举足轻重的作用。
而微积分英文版第九版课后练习题则是培养和检验读者对微积分学习的深刻理解和应用能力的重要途径。
通过对这些题目的研究和答案的掌握,可以帮助读者更好地掌握微积分学科,提升自己的学术能力和科研能力。
高三英语微积分基础单选题20题及答案

高三英语微积分基础单选题20题及答案1. In calculus, the derivative of a constant is _____.A. zeroB. oneC. itselfD. undefined答案:A。
常数的导数是零。
选项B“one”错误,常数的导数不是1。
选项C“itself”错误,常数的导数不是它本身。
选项D“undefined”错误,常数的导数是确定的,为零。
2. The process of finding the derivative is called _____.A. integrationB. differentiationC. summationD. multiplication答案:B。
求导数的过程叫做微分。
选项A“integration”是积分。
选项C“summation”是求和。
选项D“multiplication”是乘法。
3. If y = x², then the derivative of y with respect to x is _____.A. 2xB. x²C. 2x²D. x/2答案:A。
y = x²的导数是2x。
选项B“x²”错误,不是它本身。
选项C“2x²”错误,系数错误。
选项D“x/2”错误,计算错误。
4. The integral of a constant times a function is equal to the constant times the integral of the function. This is known as _____.A. the power ruleB. the product ruleC. the chain ruleD. the constant multiple rule答案:D。
常数乘以函数的积分等于常数乘以函数的积分,这被称为常数倍数法则。
微积分英文版电子档

Differential CalculusNewton and Leibniz,quite independently of one another,were largely responsible for developing the ideas of integral calculus to the point where hitherto insurmountable problems could be solved by more or less routine methods.The successful accomplishments of these men were primarily due to the fact that they were able to fuse together the integral calculus with the second main branch of calculus,differential calculus.The central idea of differential calculus is the notion of derivative.Like the integral,the derivative originated from a problem in geometry—the problem of finding the tangent line at a point of a curve.Unlile the integral,however,the derivative evolved very late in the history of mathematics.The concept was not formulated until early in the 17th century when the French mathematician Pierre de Fermat,attempted to determine the maxima and minima of certain special functions.Fermat’s idea,basically very simple,can be understood if we refer to a curve and assume that at each of its points this curve has a definite direction that can be described by a tangent line.Fermat noticed that at certain points where the curve has a maximum or minimum,the tangent line must be horizontal.Thus the problem of locating such extreme values is seen to depend on the solution of another problem,that of locating the horizontal tangents.This raises the more general question of determining the direction of the tangent line at an arbitrary point of the curve.It was the attempt to solve this general problem that led Fermat to discover some of the rudimentary ideas underlying the notion of derivative.At first sight there seems to be no connection whatever between the problem of finding the area of a region lying under a curve and the problem of finding the tangent line at a point of a curve.The first person to realize that these two seemingly remote ideas are,in fact, rather intimately related appears to have been Newton’s teacher,IsaacBarrow(1630-1677).However,Newton and Leibniz were the first to understand the real importance of this relation and they exploited it to the fullest,thus inaugurating an unprecedented era in the development of mathematics.Although the derivative was originally formulated to study the problem of tangents,it was soon found that it also provides a way to calculate velocity and,more generally,the rate of change of a function.In the next section we shall consider a special problem involving the calculation of a velocity.The solution of this problem contains all the essential fcatures of the derivative concept and may help to motivate the general definition of derivative which is given below.Suppose a projectile is fired straight up from the ground with initial velocity of 144 fee t persecond.Neglect friction,and assume the projectile is influenced only by gravity so that it moves up and back along a straight line.Let f(t) denote the height in feet that the projectile attains t seconds after firing.If the force of gravity were not acting on it,the projectile would continue to move upward with a constant velocity,traveling a distance of 144 feet every second,and at time t we woule have f(t)=144 t.In actual practice,gravity causes the projectile toslow down until its velocity decreases to zero and then it drops back to earth.Physical experiments suggest that as the projectile is aloft,its height f(t) is given by the formula.The term –16t2 is due to the influence of gravity.Note that f(t)=0 when t=0 and whent=9.This means that the projectile returns to earth after 9 seconds and it is to be understood that formula (1) is valid only for 0<t<9.The problem we wish to consider is this:T o determine the velocity of the projectile at each instant of its motion.Before we can understand this problem,we must decide on what is meant by the velocity at each instant.T o do this,we introduce first the notion of average velocity during a time interval,say from time t to time t+h.This is defined to be the quotient. Change in distance during time interval =f(t+h)-f(t)/h.ength of time intervalThis quotient,called a difference quotient,is a number which may be calculated whenever both t and t+h are in the interval[0,9].The number h may be positive or negative,but not zero.We shall keep t fixed and see what happens to the difference quotient as we take values of h with smaller and smaller absolute value.The limit process by which v(t) is obtained from the difference quotient is written symbolically as follows:The equation is used to define velocity not only for this particular example but,more generally,for any particle moving along a straight line,provided the position function f is such that the differerce quotient tends to a definite limit as h approaches zero.The example describe in the foregoing section points the way to the introduction of the concept of derivative.We begin with a function f defined at least on some open interval(a,b) on the x axis.Then we choose a fixed point in this interval and introduce the differencequotient[f(x+h)-f(x)]/h.Where the number h,which may be positive or negative(but not zero),is such that x+h also lies in(a,b).The numerator of this quotient measures the change in the function when x changes from x to x+h.The quotient itself is referred to as the average rate of change of f in the interval joining x to x+h.Now we let h approach zero and see what happens to this quotient.If the quotient.If the quotient approaches some definite values as a limit(which implies that the limit is the same whether h approaches zero through positive values or through negative values),then this limit is called the derivative of f at x and is denoted by the symbol f’(x) (read as ―f prime of x‖).Thus the formal definition of f’(x) may be stated a s follows Definition of derivative.The derivative f’(x)is defined by the equation。
英文版-微积分试卷答案-(1)

(考点:经济函数,课本4.8节。
此题型为常考题,属于送分题,大家可以做相应的4.8节的练习加以巩固)9、State the second derivative test theorem testing maximum and prove it.Suppose is continuous near c''f If and , then has a local maximum at c .'c f ()=0''c f ()< 0Proof: Because near c and so is concave downward near ''c f ()< 0f c . This means that the graph of lies below its horizontal tangent at c and so has a local maximum at c .10、Show that the equation has at most one root in the 3150x x c -+=interval.[2,2]- Suppose the equation has two root in 3150x x c -+=1212,and x x x x <the interval .12,[2,2]x x ∈-Let then there exist 3F()15x x x c =-+12F()F()=0x x =Using the Rolle’s Theorem we can know that there exist one number c can satisfy12F'(c)0(,)c x x =∈2F'()3150[2,2]x x when x =-<∈-But , Therefore, the suppose is wrong. 2F'()3150[2,2]x x when x =-<∈-Then the equation has at most one root in the interval3150x x c -+=.[2,2](考点:罗尔地理。
英文版-微积分试卷答案

1、 (1) sin 2lim x x x→∞= 0 . (2) d(arctan )x = 1/(1+x^2) . (3) 21d sin x x =⎰ -cotx .(4).2()()x n e = 泰勒展开式(书上有。
) .(5)0x =⎰ 26/3 .2、(6) The right proposition in the following propositions is ____A____.A. If lim ()x a f x →exists and lim ()x a g x →does not exist then lim(()())x af xg x →+does not exist. B. If lim ()x a f x →,lim ()x a g x →do both not exist then lim(()())x af xg x →+does not exist. C. If lim ()x a f x →exists and lim ()x a g x →does not exist then lim ()()x af xg x →does not exist. D. If lim ()x a f x →exists and lim ()x a g x →does not exist then ()lim ()x a f x g x →does not exist. (7) The right proposition in the following propositions is __A______.A. If lim ()()x af x f a →=then ()f a 'exists. B. If lim ()()x af x f a →≠ then ()f a 'does not exist. C. If ()f a 'does not exist then lim ()()x af x f a →≠. D. If ()f a 'does not exist then the cure ()y f x =does not have tangent at (,())a f a .(8) The right statement in the following statements is __D ______. A. sin lim 1x x x→∞= B. 1lim(1)x x x e →∞+= C. 11d 1x x x C ααα+=++⎰ D. 5511d d 11bb a a x y x y =++⎰⎰ (9) For continuous function ()f x , the erroneous expression in the following expressions is __C ____. A.d (()d )()d b a f x x f b b =⎰ B. d (()d )()d b af x x f a a =-⎰ C. d (()d )0d b a f x x x =⎰ D. d (()d )()()d b af x x f b f a x =-⎰ (10) The right proposition in the following propositions is ____D____. A. If ()f x is discontinuous on [,]a b then ()f x is unbounded on [,]a b .B. If ()f x is unbounded on [,]a b then ()f x is discontinuous on [,]a b .C. If ()f x is bounded on [,]a b then ()f x is continuous on [,]a b .D. If ()f x has absolute extreme values on [,]a b then ()f x is continuous on [,]a b .3、Evaluate 2011lim()x x e x x→-- 1/24.Find 0d |x y =and (0)y ''if 20x x x y y t e +=+⎰. 隐函数求导。
英文版微积分试卷答案(1)

1、(1) sin 2limx x x→∞=0 . (2)d(arctan )x =21d 1+x x (3) 21d sin x x =⎰-cot +Cx x (4).2()()x n e =22nxe (5)412d x x +=⎰26/3 2、(6) The right proposi on in the following proposi ons is ___A_____.A. If lim ()x af x →exists and lim ()x ag x →does not exist then lim(()())x af xg x →+does not exist.B. If lim ()x af x →,lim ()x ag x →do both not exist then lim(()())x af xg x →+does not exist. C. If lim ()x af x →exists and lim ()x ag x →does not exist then lim ()()x af xg x →does not exist. `D. If lim ()x a f x →exists and lim ()x ag x →does not exist then()lim ()x a f xg x →does not exist.(7) The right proposi on in the following proposi ons is __B______.A. If lim ()()x af x f a →=then ()f a 'exists. B. If lim ()()x a f x f a →≠then ()f a 'does not exist. C. If ()f a 'does not exist then lim ()()x af x f a →≠.D. If ()f a 'does not exist then the cure ()y f x =does not have tangent at (,())a f a .(8) The right statement in the following statements is ___D_____. A. sin lim1x xx x→∞= B. 1lim(1)xxx e→∞+=C. 11d 1x x x Cααα+=++⎰ D. 5511d d 11bb a a x yx y =++⎰⎰(9) For con nuous func on ()f x , the erroneous expression in the following expressions is ____D__./A.d (()d )()d ba f x x fb b =⎰ B. d (()d )()d ba f x x f a a =-⎰C. d (()d )0d ba f x x x =⎰ D. d (()d )()()d ba f x x fb f a x=-⎰(10) The right proposi on in the following proposi ons is __B______.A. If ()f x is discon nuous on [,]a b then ()f x is unbounded on [,]a b .B. If ()f x is unbounded on [,]a b then ()f x is discon nuous on [,]a b . C. If ()f x is bounded on [,]a b then ()f x is con nuous on [,]a b .D. If ()f x has absolute extreme values on [,]a b then ()f x is con nuous on [,]a b . 3、Evaluate 2011lim()xx e x x →-- 201=lim()xx e x x →--01=lim()2xx e x →-01=lim =22xx e →(考点课本节洛比达法则,每年都会有一道求极限的解答题,大多数都是用洛比达法则去求解,所以大家要注意节的内容。
微积分试卷答案

IEN INSTITUTE of MINJIANG UNIVERSITYAnswer Key and Marking CriterionClosed-book Exam Paper ASubject : Calculus Ⅰ 2013—2014 Academic Year Semester 1 Cohort & Major: AC1- 4 Teacher ’s Name: Chen LanqingPart I MCQ 12%1. A2. B3. C4. CPart Ⅱ Fill in blanks. 24%1. 21;2. 82 feet ;3. 45x +340;4. 6;5. 24y x =-+;6. 58;7. 1y =-;8. 5, 1 Part Ⅲ Find the following limits. 16%1. Solution121x x →-lim(21)13x -= 2. Solution545545652265lim lim221211x x x x x x x x x x→+∞→+∞+-+-==-+-+ 3.Solution221111lim lim 021x x x x x→+∞→+∞++==-- 4. Solution02)l i l l i m 42)42)x x x x x x →→→→+===-+=--Part ⅣDifferentiate the following functions and simplify your answers. 16% 1. Solution332324312()(1231342xf x xxxx xx x'-⎛⎫'=++⎪⎝⎭'⎛⎫=++-⎪⎝⎭=+-+2. Solution2222()[(3)1)]181)(3)181818f x x x xx x''=+=++=+-=+3. Solution12233222433110(32)(15)(32)(2)15509023()(32)3(32)x x x xx x xf xx x--+--+'⎛⎫----'===⎝++4. Solution322222411112()33111111116(1)61(1)(1)x x x xf xx x x x xx xx x x'⎛⎫''----⎛⎫⎛⎫⎛⎫⎛⎫⎛⎫' ⎪===-⎪ ⎪ ⎪ ⎪ ⎪⎪+++++⎝⎭⎝⎭⎝⎭⎝⎭⎝⎭⎝⎭--⎛⎫==⎪+⎝⎭++Part Ⅴ PROBLEM SOLVING 32% 1. Solution 12%The function f (x ) is defined for all x ≠-2 and has (0, 0) as its only intercept.2x =- is a vertical asymptote. There are no horizontal asymptotes. …….(2 pts)222(2)(2)(4)()(2)(2)x x x x x f x x x +-+'==++So ()0f x '= when 4,0x =-. We have the arrow diagram as follows :(-4, -8) and (0,0) are relative extrema. …………………….(8 pts)2243(2)(24)2(4)(2)8()(2)(2)x x x x x f x x x ++-++''==++So ()f x ''is not defined only for 2x =-. The concavity diagram can be shownbelow.Join those key features by a smooth curve.…………………………..(12 pts)2. Solution 10% a. We find that2()(0.04573)0.085c x x x x ''=++=+The estimated cost of producing the 410th unit is marginal cost (409)(0.08)(409)537.72thousand dollars.c '=+= b. The actual cost of producing the 410th unit is()22(410)(409)0.04(410)5(410)730.04(409)5(409)73c c -=++-++0.04(410409)537.76thousand dollars.=++=3. Solution 10%Let x be the number of mulch (in tons )in each shipment. The costs include:purchase cost (4,000)(20)80,000==4,000ordering cost (30)x ⎛⎫= ⎪⎝⎭storage cost (1.5)2x ⎛⎫= ⎪⎝⎭So, the total cost is120,000()80,0000.75C x x x=++ which is the function to be minimized on 14,000x ≤≤. 2120,000()0.75C x x'=-+()0C x '= when x = 4003240,000(),C x x''=so (400)0C ''> and there is a relative minimum when x = 400.C (400) = 80,600, C (1) = 200,000.75, C (4,000) = 83,030.So, the cost is minimized when 400 tons of mulch is ordered in each shipment.。
英文版-微积分试卷答案汇编

1、 (1) sin 2lim x x x→∞= 0 . (2) d(arctan )x = 1/(1+x^2) . (3) 21d sin x x =⎰ -cotx .(4).2()()x n e = 泰勒展开式(书上有。
) .(5)0x =⎰ 26/3 .2、(6) The right proposition in the following propositions is ____A____.A. If lim ()x a f x →exists and lim ()x a g x →does not exist then lim(()())x af xg x →+does not exist. B. If lim ()x a f x →,lim ()x a g x →do both not exist then lim(()())x af xg x →+does not exist. C. If lim ()x a f x →exists and lim ()x a g x →does not exist then lim ()()x af xg x →does not exist. D. If lim ()x a f x →exists and lim ()x a g x →does not exist then ()lim ()x a f x g x →does not exist. (7) The right proposition in the following propositions is __A______.A. If lim ()()x af x f a →=then ()f a 'exists. B. If lim ()()x af x f a →≠ then ()f a 'does not exist. C. If ()f a 'does not exist then lim ()()x af x f a →≠. D. If ()f a 'does not exist then the cure ()y f x =does not have tangent at (,())a f a .(8) The right statement in the following statements is __D ______. A. sin lim 1x x x→∞= B. 1lim(1)x x x e →∞+= C. 11d 1x x x C ααα+=++⎰ D. 5511d d 11bb a a x y x y =++⎰⎰ (9) For continuous function ()f x , the erroneous expression in the following expressions is __C ____. A.d (()d )()d b a f x x f b b =⎰ B. d (()d )()d b af x x f a a =-⎰ C. d (()d )0d b a f x x x =⎰ D. d (()d )()()d b af x x f b f a x =-⎰ (10) The right proposition in the following propositions is ____D____. A. If ()f x is discontinuous on [,]a b then ()f x is unbounded on [,]a b .B. If ()f x is unbounded on [,]a b then ()f x is discontinuous on [,]a b .C. If ()f x is bounded on [,]a b then ()f x is continuous on [,]a b .D. If ()f x has absolute extreme values on [,]a b then ()f x is continuous on [,]a b .3、Evaluate 2011lim()x x e x x→-- 1/24.Find 0d |x y =and (0)y ''if 20x x x y y t e +=+⎰. 隐函数求导。
微积分 高等数学 英文练习题以及答案(1)

lim
n
sin n 0. n
(n 1, 2, ) 。
n
n
(4) xn sin
n ; 2
1 证:因为 sin x 1,所以 sin n n1 有界,而 lim 0 ,根 n n
据题目已知的结论有:
则 xn 1,
(n 1, 2, ) ,lim xn 1 , 但是 lim xn 不存在。
3
Exercise 1-2 Limits of Sequences 1. Observe the behavior of the general term of each of the following sequences as n increases infinitely, determine the sequences that have limits and write out the limit if it exists: (1) xn
(7) xn cos
1 ; n
极限存在,为 1。
n 4 n 4 n 4 4 1 2 0.1 2 n n n( n 4 n) 2n
2 2
只要 n 20或n 5 即可。
2
xn a n .
(8) xn ln
1 . n
所以取 N 5 , 则当 n N , xn a 即 成立。
Xi’an Jiaotong-Liverpool University
Subgroup
Name
ID No.
1
Preliminaries: Functions 1. Find the natural domains of the following functions: (1)
AP考试模拟试题与答案1-微积分BC- AP Calculus-BC
1
14
(A) (B) 1 (C)
(D) 4 (E) 5
2
16
15. Which of the following is an equation of the line tangent to the curve with parametric equations x = 3t2 − 2, y = 2t3 + 2 at the point when t = 1?
−
d 3)
x
=
n
5x
(A) lim n→0
−3
(x
+
2)(x
−
dx 3)
−2
5x
(B) lim n→− 3 + n
(x
+
2)(x
−
dx 3)
n
5x
(C) lim n→− 2−
− 3 (x + 2)(x − 3) d x
n
5x
(D) lim n→− 3
ห้องสมุดไป่ตู้
−3
(x
+
2)(x
−
dx 3)
−2
5x
(E) lim n→0 n
III. f < 0 on (0, b)
(A) III only (B) I and II only
(C) II and III only (D) I and III only (E) I, II, and III
y f
a
0
x b
Figure 1T-3
7.
∞
1
=
n = 1 (2n − 1)(2n + 1)
微积分试卷-英文版3

⑵d(arctan x) _ 1/(1+x A2) _____ .1(3) — dx -cotx .sin x(4). (e2x)(n)泰勒展开式(书上有。
)26/3(6) The right propositi on in the follow ing propositi ons is A .A. If lim f (x) exists and lim g(x) does not exist then lim( f(x) g(x)) does notx a x a x aexist.B. If lim f (x), lim g(x)do both not exist then lim( f (x) g(x)) does not exist.x a x a x aC. If lim f (x) exists and lim g(x)does not exist then lim f (x)g(x) does notx a x a x aexist.D. If lim f (x) exists and lim g(x)does not exist thenx a x aman Xf(x) does not exist.g(x)(7)The right propositi on in the followi ng propositi ons isA. If lim f (x) f (a)then f (a) exists.x aB. If lim f (x) f (a) then f (a) does not exist.x aC. If f (a) does not exist then lim f (x) f (a).x aD. If f (a) does not exist then the cure y f (x) does not have tangent at (a, f (a)).(8) The right statement in the following statements is __A. lim 叱1x x1 B. lim(1 x),xC. x dx —x 1 C1 D.a1 x5(10) The right propositi on in the followi ng propositi ons is _ ____ DA. If f (x) is discontinuous on [a,b]then f(x)is unbounded on [a,b].B. If f (x) is unbounded on [a,b] then f (x) is discontinuous on [a, b].C. If f (x) is bounded on [a,b] then f (x) is continuous on [a,b].求导为2*x*y(x) (y(x)就是y) 5、Find 严吟 dx . x 2(1 x 2)arcta nx/x A2-arcta nx/(1+x A2)=-(arctanx)/x+ / dx/[x(1+xA2)]=-(arctanx)/x+-xyd ((1+xA2)+ / dx/x=-(arctanx)/x- (1/2) / d(1+xA2)/(1+xA2)+ / dx/x=-(arcta nx)/x-(1/2)l n(1+xA2)+l n|x|+Cexpressi ons is _ C ___“ d bA. (a f(x)dx) f (b) db ad bC. ( f (x)dx) 0 dx aba f(x)dx)b f (x)dx)af (a) f(b) f(a)D. If f (x) has absolute extreme values on [a,b] then f(x) is continuouson [a,b].3、Evaluate 1/24 . Find dy|x °and y (0) if x20 yGt)dte x .隐函数求导。
大一上学期微积分试卷Calculus(I)期中考试卷及答案

------------------------- 赠予 ------------------------【名师心得】1. 因材施教,注重创新。
所讲授的每门课程应结合不同专业、不同知识背景的学生来调整讲授的内容和方法。
不仅重视知识的传授,更要重视学生学习能力、分析和解决问题能力的培养,因为这些才是学生终生学习的根本。
注重教学创新,不仅体现在教学模式、教学方法方面,更主要的是体现在内容的创新与扩充、实践环节的同步改革上。
2. 学高为师,身正为范。
不但要有崇高的师德,还要有深厚而扎实的专业知识。
要做一名让学生崇拜的师者,就要不断的更新知识结构,拓宽知识视野,自己不断的钻研学习,加强对教材的驾御能力才能提高自己的教学方法,才能在学生心目中树立起较高的威信。
因此,必须树立起终身学习的观念,不断的更新知识、总结经验,取他人之长来补己之短,才1江西财经大学2013级期中(上)试卷(参考答案) 课程代码:12003(A ) 授课课时:48 考试时间:110分钟 课程名称:Calculus (I) 适用对象:13级AR1、AR6等班级的学生 试卷命题人: . 试卷审核人: .1. Full in the blank of each statement in the following five statements such that the statement is right. Then write the corresponding answer on the answer book by the title number. You can be gained 3 points for the right thing on per blank.(1) 2(2)x x '+=________.(2) 22121lim 1x x x x →++=+_________. (3) 2d (log )d e x x=________. (4) 226lim 345x x x x →±∞=++________.------------------------- 赠予 ------------------------【名师心得】1. 因材施教,注重创新。
高三英语微积分基础单选题20题

高三英语微积分基础单选题20题1. In the function f(x) = 3x^2 + 5x - 2, the derivative of f(x) at x = 1 is:A. 11B. 8C. 10D. 12答案:A。
本题考查导数的基本运算。
首先对函数f(x) 求导得到f'(x) = 6x + 5,将x = 1 代入f'(x),得到f'(1) = 6×1 + 5 = 11。
选项B 计算错误;选项C 和D 也是计算错误。
2. If the integral of f(x) from 0 to 2 is 10, and the integral of f(x) from0 to 1 is 4, then the integral of f(x) from 1 to 2 is:A. 6B. 8C. 14D. 16答案:A。
根据定积分的性质,从a 到b 的积分等于从a 到c 的积分加上从 c 到 b 的积分。
所以从1 到 2 的积分为从0 到 2 的积分减去从0 到1 的积分,即10 - 4 = 6。
选项B、C、D 计算错误。
3. The slope of the tangent line to the curve y = x^3 at the point (1, 1) is:A. 1B. 3C. 2D. 4答案:B。
对y = x^3 求导得y' = 3x^2,将x = 1 代入得斜率为3×1^2 = 3。
选项A、C、D 计算错误。
4. The area under the curve y = 2x + 1 from x = 1 to x = 3 is:A. 10B. 12C. 8D. 14答案:A。
先求出定积分,∫(2x + 1)dx = x^2 + x,代入上限3 和下限1,得到(3^2 + 3) - (1^2 + 1) = 12 - 2 = 10。
微积分试卷及答案4套

微积分试卷及答案4套微积分试题(A卷)一.填空题(每空2分,共20分)1.已知$\lim\limits_{x\to1^+}f(x)=A$,则对于$\forall\epsilon>0$,总存在$\delta>0$,使得当$x\to1^+$时,恒有$|f(x)-A|<\epsilon$。
2.已知$\lim\limits_{n\to\infty}\dfrac{a_n^2+bn+5}{n^2+3n-2}=2$,则$a=1$,$b=3$。
3.若当$x\to x_0$时,$\alpha$与$\beta$是等价无穷小量,则$\lim\limits_{x\to x_0}\dfrac{\alpha-\beta}{\beta}=0$。
4.若$f(x)$在点$x=a$处连续,则$\lim\limits_{x\toa}f(x)=f(a)$。
5.函数$f(x)=\ln(\arcsin x)$的连续区间是$(0,1]$。
6.设函数$y=f(x)$在$x$点可导,则$\lim\limits_{h\to0}\dfrac{f(x+3h)-f(x)}{h}=3f'(x)$。
7.曲线$y=x^2+2x-5$上点$M$处的切线斜率为6,则点$M$的坐标为$(-1,2)$。
8.$\dfrac{d(xf'(x))}{dx}=xf''(x)+2f'(x)$。
9.设总收益函数和总成本函数分别为$R=24Q-2Q^2$,$C=Q+5$,则当利润最大时产量$Q=6$。
二.单项选择题(每小题2分,共18分)1.若数列$\{x_n\}$在$a$的$\epsilon$邻域$(a-\epsilon,a+\epsilon)$内有无穷多个点,则(B)数列$\{x_n\}$极限存在,且一定等于$a$。
2.设$f(x)=\arctan\dfrac{2}{x-1}$,则$x=1$为函数$f(x)$的(A)可去间断点。
微积分 高等数学 英文练习题以及答案(2)
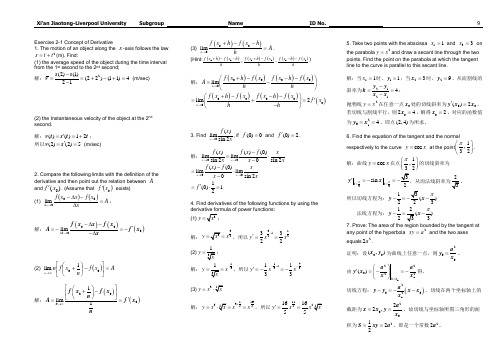
Exercise 2-1 Concept of Derivative1. The motion of an object along the s -axis follows the law2s t t =+(m). Find:(1) the average speed of the object during the time interval from the 1st second to the 2nd second; 解:2(2)(1)(22)(11)421s s v -==+-+=- (m/sec)(2) the Instantaneous velocity of the object at the 2nd second.解:()()12v t s t t '==+; 所以(2)(2)5v s '== (m/sec)2. Compare the following limits with the definition of the derivative and then point out the relation between A and 0()f x '. (Assume that ()0x f ' exists) (1) ()()A xx f x x f x =∆-∆-→∆000lim ;解:()()()0000lim x fx x fx A f x x∆→-∆-'=-=--∆(2) ()A x f n x f n n =⎥⎦⎤⎢⎣⎡-⎪⎭⎫ ⎝⎛+∞→001lim解:()()0001lim1n f x f x n A f x n→∞⎡⎤⎛⎫+- ⎪⎢⎥⎝⎭⎣⎦'==(3) ()()00limh fx h fx h A h→+--=.(Hint:()()()()()()000000f x h f x h f x h f x f x h f x hhh+--+---=-)解:()()()()00000lim →+---⎛⎫=- ⎪⎝⎭h f x h f x f x h f x A h h ()()()()()000000lim 2→+---⎛⎫'=+= ⎪-⎝⎭h f x hf x f x h f x f x h h3. Find 0()lim sin 2→x f x x, if (0)0f = and (0)2f '=.解:00()()(0)lim lim sin 20sin 2→→-=⋅-xx f x f x f xx x x00()(0)lim lim 0sin 21(0)12→→-=⋅-'=⋅=x x f x fxx x f4. Find derivatives of the following functions by using the derivative formula of power functions:(1)y =解:32==y x ,所以311223322-'==y x x(2)y =; 解:13-==y x,所以141331133---'=-=-y xx(3)3y x =解:1163355+===y x x x,所以1125161655'==y x x5. Take two points with the abscissa 11=x and 33=x on the parabola 2x y =and draw a secant line through the two points. Find the point on the parabola at which the tangent line to the curve is parallel to this secant line.解:当11=x 时,11y =;当23x =时,29y =。
AP 微积分BC 选择题样卷一

AP Calculus Practice ExamBC Version - Section I - Part ACalculators ARE NOT Permitted On This Portion Of The Exam28 Questions - 55 Minutes1) GivenFind dy/dx.a)b)c)d)e)2) Give the volume of the solid generated by revolving the region bounded by the graph of y = ln(x), the x-axis, the lines x = 1 and x = e, about the y-axis.a)b)c)d)e)3) The graph of the derivative of f is shown below.Find the area bounded between the graph of f and the x-axis over the interval [-2,1], given that f(0) = 1.a)b)c)d)e)4) Determine dy/dt, given thatanda)b)c)d)e)5) The functionis invertible. Give the slope of the normal line to the graph of f -1 at x = 3.a)b)c)d)e)6) Determinea)b)c)d)e)7) Give the polar representation for the circle of radius 2 centered at ( 0 , 2 ).a)b)c)d)e)8) Determinea)b)c)d)e)9) Determinea)b)c)d)e)10) Give the radius of convergence for the seriesa)b)c)d)e)11) Determinea)b)c)d)e)12) The position of a particle moving along the x-axis at time t is given byAt which of the following values of t will the particle change direction I) t = 1/8II) t = 1/6III) t = 1IV) t = 2a) I, II and IIIb) I and IIc) I, III and IVd) II, III and IVe) III and IV13) Determinea)b)c)d)e)14) Determine the y-intercept of the tangent line to the curveat x = 4.a)b)c)d)e)15) The function f is graphed below.Give the number of values of c that satisfy the conclusion of the Mean Value Theorem for derivatives on the interval [2,5].a)b)c)d)e)16) Give the average value of the functionon the interval [1,3].a)b)c)d)e)17) A rectangle has both a changing height and a changing width, but the height and width change so that the area of the rectangle is always 20 square feet. Give the rate of change of the width (in ft/sec) when the height is 5 feet, if the height is decreasing at that moment at the rate of 1/2 ft/sec.a)b)c)d)e)18) The graph of the derivative of f is shown below.Give the number of values of x in the interval [-3,3] where the graph of f has inflection.a)b)c)d)e)19) A rectangle has its base on the x-axis and its vertices on the positive portion of the parabolaWhat is the maximum possible area of this rectanglea)b)c)d)e)20) Computea)b)c)d)e)21) Determinea)b)c)d)e)22) Determinea)b)c)d)e)23) Give the exact value ofa)b)c)d)e)24) Determinea)b)c)d)e)25) Give the derivative ofa)b)c)d)e)26) Give the first 3 nonzero terms in the Taylor series expansion about x = 0 for the functiona)b)c)d)e)27) Determinea)b)c)d)e)28) Which of the following series converge(s)a) B onlyb) A, B and Cc) B and Cd) A and Be) A and C1) d)2) e)3) b)4) b)5) e)6) a)7) c)8) c)9) b)10) d)11) c)12) c)13) c)14) e)15) a)16) d)17) a)18) b)19) a)20) d)21) b)22) b)23) a)24) c)25) d)26) b)27) b)28) c)。
ap微积分ab试卷

ap微积分ab试卷English Answer:1. The derivative of f(x) = x^3 + 2x^2 5x + 1 is f'(x)= 3x^2 + 4x 5.2. The integral of f(x) = x^3 + 2x^2 5x + 1 is F(x) = (x^4)/4 + (2x^3)/3 (5x^2)/2 + x + C.3. The limit of (x^2 1)/(x 1) as x approaches 1 is 2.4. The equation of the tangent line to the graph of f(x) = x^3 + 2x^2 5x + 1 at the point (1, -3) is y = 6x 9.5. The area under the curve of f(x) = x^3 + 2x^2 5x + 1 from x = 0 to x = 2 is 9.6. The volume of the solid generated by rotating the region bounded by the curves y = x^2 and y = 4 about the x-axis is (64pi)/5.7. The work done by the force F(x) = x^2 + 2x from x = 0 to x = 2 is 14/3.8. The center of mass of a thin wire of length L with density p(x) = x is at x = L/2.9. The moment of inertia of a thin rod of length L about an axis perpendicular to the rod and passing through one end is (ML^2)/12.10. The Laplace transform of f(t) = e^(-t) is F(s) = 1/(s + 1).中文回答:1. f(x) = x^3 + 2x^2 5x + 1 的导数为 f'(x) = 3x^2 + 4x 5。
英文版微积分试卷答案(1)

英文版微积分试卷答案(1)1、 (1) sin 2limx x x→∞= 0 . (2) d(arctan )x = 21d 1+xx(3)21d sinx x=-cot +Cx x(4).2()()x n e = 22n x e .(5)0x =?26/32、(6) The right proposition in the following propositions is ___A_____.A. If lim ()x af x →exists and lim ()x ag x →does not exist then lim (()())x af xg x →+does not exist.B. If lim ()x af x →,lim ()x ag x →do both not exist then lim (()())x af xg x →+does not exist.C. If lim ()x af x →exists and lim ()x ag x →does not exist then lim ()()x af xg x →does not exist.D. If lim ()x af x →exists and lim ()x ag x →does not exist then ()lim()x af xg x →does not exist.(7) The right proposition in the following propositions is __B______.A. If lim ()()x af x f a →=then ()f a 'exists.B. If lim ()()x af x f a →≠ then ()f a 'does not exist.C. If ()f a 'does not exist then lim ()()x af x f a →≠.D. If ()f a 'does not exist then the cure ()y f x =does not have tangent at (,())a f a .(8) The right statement in the following statements is ___D_____.A. sin lim1x x x→∞= B. 1lim (1)x x x e →∞+=C.11d 1x x xCααα+=++?D.5511d d 11b b aax yxy=++?(9) For continuous function ()f x , the erroneous expression in the following expressionsis ____D__. A.d (()d )()d ba f x x fb b =? B.d (()d )()d baf x x f a a =-?C.d (()d )0d baf x x x=? D.d (()d )()()d baf x x f b f a x=-?(10) The right proposition in the following propositions is __B______.A. If ()f x is discontinuous on [,]a b then ()f x is unbounded on [,]a b .B. If ()f x is unbounded on [,]a b then ()f x is discontinuous on [,]a b .C. If ()f x is bounded on [,]a b then ()f x is continuous on [,]a b .D. If ()f x has absolute extreme values on [,]a b then ()f x is continuous on [,]a b . 3、Evaluate 211lim ()xx e xx→--21=lim ()xx e xx→--01=lim ()2xx e x→-01=lim=22xx e→(考点课本4.4节洛比达法则,每年都会有一道求极限的解答题,大多数都是用洛比达法则去求解,所以大家要注意4.4节的内容。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
1、 (1) sin 2limx x x→∞= 0 . (2) d(arctan )x = 21d 1+xx(3)21d sinx x=⎰-cot +Cx x(4).2()()x n e = 22n x e .(5)0x =⎰26/32、(6) The right proposition in the following propositions is ___A_____.A. If lim ()x af x →exists and lim ()x ag x →does not exist then lim (()())x af xg x →+does not exist.B. If lim ()x af x →,lim ()x ag x →do both not exist then lim (()())x af xg x →+does not exist.C. If lim ()x af x →exists and lim ()x ag x →does not exist then lim ()()x af xg x →does not exist.D. If lim ()x af x →exists and lim ()x ag x →does not exist then ()lim()x af xg x →does not exist.(7) The right proposition in the following propositions is __B______.A. If lim ()()x af x f a →=then ()f a 'exists.B. If lim ()()x af x f a →≠ then ()f a 'does not exist.C. If ()f a 'does not exist then lim ()()x af x f a →≠.D. If ()f a 'does not exist then the cure ()y f x =does not have tangent at (,())a f a .(8) The right statement in the following statements is ___D_____.A. sin lim1x x x→∞= B. 1lim (1)x x x e →∞+=C.11d 1x x xCααα+=++⎰D.5511d d 11b b aax yxy=++⎰⎰(9) For continuous function ()f x , the erroneous expression in the following expressionsis ____D__. A.d (()d )()d ba f x x fb b =⎰ B.d (()d )()d baf x x f a a =-⎰C.d (()d )0d baf x x x=⎰ D.d (()d )()()d baf x x f b f a x=-⎰(10) The right proposition in the following propositions is __B______.A. If ()f x is discontinuous on [,]a b then ()f x is unbounded on [,]a b .B. If ()f x is unbounded on [,]a b then ()f x is discontinuous on [,]a b .C. If ()f x is bounded on [,]a b then ()f x is continuous on [,]a b .D. If ()f x has absolute extreme values on [,]a b then ()f x is continuous on [,]a b . 3、Evaluate 211lim ()xx e xx→--21=lim ()xx e xx→--01=lim ()2xx e x→-01=lim=22xx e→(考点课本4.4节洛比达法则,每年都会有一道求极限的解答题,大多数都是用洛比达法则去求解,所以大家要注意4.4节的内容。
注意洛比达法则的适用范围。
)4.Find 0d |x y =and (0)y ''if 2x xx y y t e+=+⎰.2'()'x xx y y t e +=+⎰()1'2()'2()1xxy x y x e y x y x e +=⋅+⇒=⋅+-(20(0)1)0x dyy e dx dx==⋅⋅+-=''(2()1)'2()2'()xxy x y x e y x xy x e=⋅+-=++2-(0)0-01x x y y t e x y e =+⇒=+=⎰''02(0)20'(0)=3y y y e =+⋅+()(考察微积分基本定理与微分,书上5.3节)5、 Find 22arctan d (1)x x x x +⎰=22221)arctan d (1)x x xxx x +-+⎰(22arctan arctan =d d (1)x x x xxx -+⎰⎰-12311=-arctan +d arctan +2x x x x x x-⎰22-1221++1=-arctan +d arctan 1+2x xx x x x x x -⎰() -12211=-arctan +d d arctan 1+2x x x x x x x x --⎰⎰() -12211=-arctan +In In 1+arctan 22x x x xx ---121=-arctan +Inarctan +C 2x x x -(凑微分求不定积分,积分是微积分的重点及难点,大家一定要掌握透彻。
)6、 Given that 22()1xf x x =+.(1) Find the intervals on which ()f x is increasing or decreasing. 22’22212()1x x x xf x x +-⋅=+()()2221xx =+()When ’()00f x x >⇒>’()00f x x <⇒< Therefore, the increasing interval is ()0+∞,, the decreasing interval is ()0-∞,(2) Find the local maximum and minimum values of ()f x’()00f x x =⇒= The function is increasing in interval()0+∞,, decreasing ininterval ()0-∞,, therefore, the function exist the local minimum value, it is ()0f x =(3)Find the intervals of concavity and the inflection points.'22224222242422181642''()111x x x x x x f x x x x +-+--+⎛⎫=== ⎪+++⎝⎭()()()()()4224642''()0133x x f x x x x --+=>⇒><-+()4224642''()000133x x f x x or x x --+=<⇒-<<<<+()1''(334f f =-Therefore, the concave upward intervalare 3⎛-∞-⎪⎝⎭,,3⎛⎫+∞ ⎪ ⎪⎝⎭, the concave downwardinterval are -03⎛⎫ ⎪ ⎪⎝⎭,03⎛⎫ ⎪ ⎪⎝⎭,and the inflection points are 1-34⎛⎫ ⎪ ⎪⎝⎭,,134⎛⎫⎪ ⎪⎝⎭,(4) Find the asymptote lines of the cure ()y f x =2221lim=1111+x xx x→∞=+T herefore, the liney = 1 is a horizontal asymptote(考点:4.3节,4.5、4.6节。
近几年经常会考一道作图题。
这种题目应该在注意的点主要包括函数的定义域,对称性,增减区间,极值点,凹凸性,拐点,以及渐近线等。
大家参照课本的4.5节进行作图)7、Let R be the region bounded by the curve 1y x=, and the line y x = and 2x =.(a)Evaluate the area of the region R. R=211x dx x-⎰2211=In 2x x-2211=2In21In122⋅--⋅+3=In22-(b)Find the volume of the solid generated by revolving the R about the y -axis .V=21212121 4) y dy dyyππ-+-⎰⎰((12311211443x yy yπ⎛⎫ ⎪=-++⎪⎝⎭33111142241141423312π⎛⎫=⋅-⋅-⋅+⋅+⋅+-⋅- ⎪⎝⎭ 83π=(考点:求面积以及体积,课本6.1、6.2节。
这类题目是常考题,较简单。
望同学一定要做相应的题目加以巩固。
)8、 Determine the production level that will maximize the profit for a company withcost and demand functions23()1450360.580.001C x x x x =+-+and ()600.01p x x =-.Solution 2()(600.01)600.01R x x x x x =-=-223()()()600.01(1450360.580.001)P x R x C x x x x x x =-=--+-+320.0010.57241450x x x =-++-'2()0.003 1.142P x x x =-++Let '()040020P x x or x =⇒==- since x>0, then x=400''()0.006 1.14P x x =-+When x=400 ''()0.006400 1.14 1.260P x =-⋅+=-<32(400)0.0014000.5740024400145035350P =-⋅+⋅+⋅-=Therefore, when the production level is 400 that will maximize the profit 35350(考点:经济函数,课本4.8节。
