期权期货及其衍生产品约翰赫尔官方课件

合集下载

[考研专业课课件] 赫尔《期货、期权及其他衍生产品》课件第34章实物期权

常数）。定义V为两年后所报出的每平方英尺写字
楼空间的费用。假设每年都是预先交付租金。期权的收益是 100000 Amax（V-35，0）
其中A是由下式所给出的摊还因子
A 1 1 e 0.051 1 e 0.052 1 e 0.053 1 e 0.054 4.5355
ˆ V 30e 0.062 33.82 E
在风险中性世界里收益的期望值是110.15万
美元期权的价值为150.14e-0．05×2=135.86万美元结论：为这个期权支付100万美元是划算的。
34．3 估计风险市场价格
当一个特定变量存在历史数据时，可以用资本资产定价模型来估计它的风险市场价格。考虑一个仅依赖于这个变量的投资资产。定义： μ——投资资产收益率的期望； σ——投资资产收益的波动率； λ——变量的风险市场价格； ρ——变量的百分比变化与一个包含广泛股
考虑一项费用为1亿美元而延续5年的投资。
预期每年的现金流入估计为2500万美元。如果经过风险调整后的贴现率是12％（连续复利），那么投资的净现值（以百万计）是
100 25e 0.121 25e 0.122 25e 0.123 25e 0.124 25e 0.125 11.53
2．如何计算适用于基本项目（即没有隐含期
权的项目）贴现率。
34．2 风险中性定价的推广
在27．1节里，定义一个变量θ的风险市场价格为
r
（34-1）
对任何仅依赖于θ的可交易证券都会得到同样的风险市场价格λ。假设某个实物资产依赖于一些变量 θi（i=l，2，…）。令mi和σi分别为θi的增长
率期望和波动率，于是
d i
i
mi dt si dzi

期权期货及其衍生产品约翰赫尔官方课件97522共21页

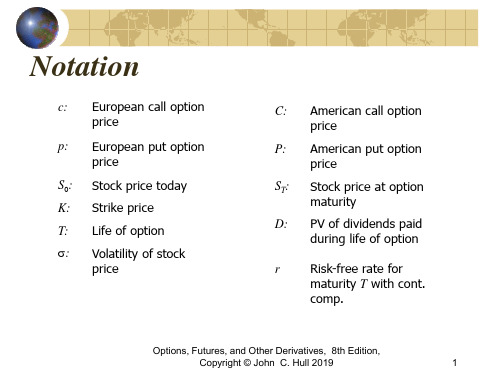
Notation
c: European call option price
p: European put option price
S0: Stock price today K: Strike price T: Life of option s: Volatility of stock
price
C: American call option price
ST > K ST − K
K ST 0 ST ST
ST < K 0 K K
K− ST ST K
Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
9
The Put-Call Parity Result (Equation
P: American put option price
ST: Stock price at option maturity
D: PV of dividends paid during life of option
r Risk-free rate for maturity T with cont. comp.
(Equation 10.5, page 221)
p Ke -rT–S0
Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
7
Put-Call Parity: No Dividends
Cc Pp
Options, Futures, and Other Derivatives, 8th Edition,

期权期货及其衍生产品约翰赫尔官方课件共29页

Copyright © John C. Hull 2019
2
Mechanics of Put Futures Option
When a put futures option is exercised the holder acquires
A short position in the futures A cash amount equal to the excess of the strike price over the futures price at the time of the most recent settlement
Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
1
Mechanics of Call Futures Options
When a call futures option is exercised the holder acquires
A long position in the futures A cash amount equal to the excess of the futures price at the time of the most recent settlement over the strike price
Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
6
Potential Advantages of Futures Options over Spot Options

[考研专业课课件] 赫尔《期货、期权及其他衍生产品》课件第28章利率衍生产品：标准市场模型

B T
和
d2 d1 B T
又如5．5节所述，FB可以利用以下公式来计算
B0 I FB P 0, T
（28-3）
B0——债券在0时刻的价格
I——期权有效期内债券所支付息票的贴现值
注意：公式中，即期债券价格与远期债券价
格均为现金价格（cash price），而非报价（ quoted price）。式（28-1）和式（28-2）中的执行价格K是现金价格，实际中通常执行价格为报价，所以K应等于执行价格+在到期日时的应计利息。
【例28-2】考虑一个10个月期的欧式看涨期
权，标的资产是期限为9.75年，面值为1 000美元的债券（当期权到期时，该债券的剩余期限为8年零11个月）。假设，债券的当前现金价格为960美元执行价格为1 000美元 10个月期的无风险利率为每年10％债券远期价格的波动率为每年9％
债券券息率为10％，每半年支付一次
2．对于债券持有人而言，由于看跌期权增加
了债券本身的价值，附有可退还条款的债券比没有这种条款的债券收益率要低。
应用：贷款、储蓄、贷款许诺
1．假定某金融机构的5年定期存款可以被提前提取而没有任何惩罚，那么它就包含一个债券美式看跌期权（存款是一个投资者有权在任何时刻以其面值卖给金融机构的债券）。 2．在贷款和有抵押贷款中可以提前付清的权利也是关于债券的看涨期权。
=0.9200，σB=0.09及T=10/12，由（28-1），看
涨期权的价格为9.49美元。
（b）如果执行价格是报价
因为期权的到期日是息票支付后的一个月，K 中必须再加上一个月的累计利息， K=1000+100×0.08333=1008.33 FB =939.68，P（0，T）=0.9200，σB=0.09， T=0.8333）由式（28-1），看涨期权价格为7.97美元。

期权期货及其衍生产品,约翰赫尔官方课件

Options, Futures, and Other Derivatives, 8th Edition, Copyright © John C. Hull 2012
14
Final Tree; Fig 33.2
E 44.35 B 30.49 A 20.00 C 21.56 D 15.25 F 31.37 G 22.18 H 15.69 I 11.10 J 45.68 K 32.30 L 22.85 M 16.16 N 11.43
dS q* (t ) a ln S dt sdz S

Assume a = 0.1, s = 0.2, and Dt = 1 year
Options, Futures, and Other Derivatives, 8th Edition, Copyright © John C. Hull 2012
H 0.2217 0.6566 0.1217
I 0.0867 0.0266 0.8867
Options, Futures, and Other Derivatives, 8th Edition, Copyright © John C. Hull 2012
13
Determining q(t)
The nodes on the tree are moved so that the expected commodity price equals the futures price Assume that the one-year, two-year and three-years futures price for the commodity are $22, $23, and $24, respectively

期权期货及其衍生产品约翰赫尔官方课件-PPT精选文档

Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
2
Day Count Conventions in the U.S. (Page 129)
Treasury Bonds: Actual/Actual (in period)
Bond: 8% Actual/ Actual in period.
4% is earned between coupon payment dates. Accruals on an Actual basis. When coupons are paid on March 1 and Sept 1, how much interest is earned between March 1 and April 1?
Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
10
Conversion Factor
Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
4
Examples continued
T-Bill: 8% Actual/360:
8% is earned in 360 days. Accrual calculated by dividing the actual number of days in the period by 360. How much interest is earned between March 1 and April 1?

期权期货及其衍生产品约翰赫尔官方课件97522PPT精品文档21页

Copyright © John C. Hull 2019
2
Effect of Variables on Option Pricing (Table 10.1, page 215)
Variable
c
p
C
P
S0
+
−
+
−
K
−
+
−
+
T
?
?
+
+
s
+
+
+
+
r
+
−
+
Байду номын сангаас
−
D
−
+
−
+
Options, Futures, and Other Derivatives, 8th Edition,
Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
11
Arbitrage Opportunities
Suppose that
c= 3 T = 0.25 K =30
Copyright © John C. Hull 2019
5
Lower Bound for European Call Option Prices; No Dividends (Equation 10.4, page 220)
c S0 –Ke -rT
Options, Futures, and Other Derivatives, 8th Edition,

期货期权及其衍生品配套课件(全34章)Ch03.ppt

Cost of Asset=S2 – (F2 – F1) = F1 + Basis
Options, Futures, and Other Derivatives, 7th International
Edition, Copyright © John C. Hull 2008
7
Short Hedge
Options, Futures, and Other Derivatives, 7th International
Edition, Copyright © John C. Hull 2008
8
Choice of Contract
Choose a delivery month that is as close as possible to, but later than, the end of the life of the hedge When there is no futures contract on the asset being hedged, choose the contract whose futures price is most highly correlated with the asset price. This is known as cross hedging.
Options, Futures, and Other Derivatives, 7th International
Edition, Copyright © John C. Hull 2008
6
Long Hedge
We define
F1 : Initial Futures Price F2 : Final Futures Price S2 : Final Asset Price If you hedge the future purchase of an asset by entering into a long futures contract then

赫尔《期权期货及其他衍生产品》第1章(第八版)讲述

期权、期货及其他衍生产品（第八版） Copyright © John C. Hull 2012
32
对冲基金( 见业界事例1-2，p8)
• 对冲基金受到的约束与共同基金不同，一般不对外公布持有的证券组合。
• 共同基金必须
– 披露投资策略 – 在任意时刻允许份额赎回 – 杠杆率受到限制 – 不能持有空头头寸
20
2. 石油:另外一种套利机会?
假定:
- 石油的即期价格为95美元 - 1年期原油期货的标价为80美元 - 1年期的美元利率为 5% - 原油的储存成本为每年2%
是否存在套利机会?
期权、期货及其他衍生产品（第八版） Copyright © John C. Hull 2012
21
期权
• 看涨期权：其持有者有权在将来某一特定时间以某一确定价格（执行价格）买入某种资产。
卖出价 1.4411 1.4413 1.4415 1.4422
期权、期货及其他衍生产品（第八版） Copyright © John C. Hull 2012
8
远期价格
• 合约的远期价格是今天约定的合约支付价格（使合约价值为零的支付价格）；
• 对不同期限的远期合约而言，远期价格也不同（如表1-1所示）。
期权、期货及其他衍生产品（第八版） Copyright © John C. Hull 2012
34
期权、期货及其他衍生产品（第八版） Copyright © John C. Hull 2012
23
表1-2 谷歌股票看涨期权在2010年6月15日的价格
(P6)
执行 2010年7月
价格
买入价
2010年7月卖出价
2010年9月买入价

期权、期货及其他衍生产品第9版-赫尔】Ch(9)幻灯片PPT

Options, Futures, and Other Derivatives, 9th Edition, Copyright ©
John C. Hull 2014
4
Historical Simulation to Calculate the One-Day VaR
Create a database of the daily movements in all market variables. The first simulation trial assumes that the percentage changes in all market variables are as on the first day The second simulation trial assumes that the percentage changes in all market variables are as on the second day and so on
Let vi be the value of a variable on day i
There are 500 simulation trials
The ith trial assumes that the value of the market
variable tomorrow is
v500
vi vi1
期权、期货及其他衍生产品第9 版-赫尔】Ch(9)幻灯片PPT
本PPT课件仅供大家学习使用请学习完及时删除处理谢谢！
Options, Futures, and Other Derivatives, 9th Edition,
Copyright © John C. Hull 2014

[考研专业课课件] 赫尔《期货、期权及其他衍生产品》课件第1章导言

国内外经典教材名师讲堂
《期权、期货及其他衍生产品》
第1章导言
第一部分本章要点
●衍生市场的发展 ●远期、期货和期权市场的发展
●市场参与者如何使用衍生产品
第二部分重难点导学
1．1 1．2 衍生市场的发展远期、期货和期权市场的发展
1．2．1 远期合约
远期合约：在将来某一指定时刻以约定价格买入或卖出某一产品的合约。远期合约是在场外交易市场中金融机构之间或金融机构与其客户之间的交易。
1.441的价格卖出3000万英镑。
实际效果：卖出英镑而收入美元,数量为 43230000美元。注意，一个公司选择不对冲可能会比选择对冲的盈利效果更好，也有可能更差。为什么？
例5：采用期权对冲。考虑某投资者在某年5 月份拥有1000股微软股票的情形。股票价格为每股28美元。投资者十分担心今后2个月股票下跌，所以想买入期权保护。
图1-2
交易产生的净盈利（假定为欧式期权）
1．3 交易员的种类
交易员可以分为三大类：对冲者：采用衍生产品合约来减少自身面临的由于市场变化而产生的风险。投机者：利用这些产品对今后市场变量的走向下赌注。套利者：采用两个或更多相互抵消的交易来锁定盈利。
1．3．1
对冲者
例3：利用远期对冲。假定今天是2010年5月 24日，一家美国的进口公司ImpoaCo得知在2010年
期权合约：赋予持有者去做某事的权利的合约。看涨期权的持有者有权在将来某一特定时间以某一确定价格买入某种资产；看跌期权的持有者有权在将来某一特定时间以某一确定价格卖出某种资产。美式期权是指在到期前的任何时刻，期权持
有人均可以行使期权；欧式期权是指期权持有人
只能在到期这一特定时刻行使期权。

期权期货及其衍生产品约翰赫尔官方课件

Options, Futures, and Other Derivatives, 8th Edition, Copyright © John C. Hull 2012
9
First Scenario for the Example:
Table 18.2 page 384
Week 0 Stock price 49.00 Delta 0.522 Shares purchased 52,200 Cost (‘$000) 2,557.8 Cumulative Cost ($000) 2,557.8 Interest 2.5
Relationship Between Delta, Gamma, and Theta (page 393)
For a portfolio of derivatives on a stock paying a continuous dividend yield at rate q it follows from the Black-ScholesMerton differential equation that
Options, Futures, and Other Derivatives, 8th Edition, Copyright © John C. Hull 2012
11
Theta
Theta (Q) of a derivative (or portfolio of derivatives) is the rate of change of the value with respect to the passage of time The theta of a call or put is usually negative. This means that, if time passes with the price of the underlying asset and its volatility remaining the same, the value of a long call or put option declines

期权期货及其衍生产品约翰赫尔官方课件

c S0e qT Ke rT
Lower Bound for puts
p Ke
Put Call Parity
rT
S0e
qT
c Ke rT p S0e qT
c Ke rT p F0e rT
11
Options, Futures, and Other Derivatives, 8th Edition, Copyright © John C. Hull 2012
Options, Futures, and Other Derivatives, 8th Edition, Copyright © John C. Hull 2012
5
Example 2
Portfolio has a beta of 2.0 It is currently worth $500,000 and index stands at 1000 The risk-free rate is 12% per annum The dividend yield on both the portfolio and the index is 4% How many put option contracts should be purchased for portfolio insurance?
Options, Futures, and Other Derivatives, 8th Edition, Copyright © John C. Hull 2012 8
European Options on Assets Providing a Known Yield
We get the same probability distribution for the asset price at time T in each of the following cases: 1. The asset starts at price S0 and provides a yield = q 2. The asset starts at price S0e–qT and provides no income

[考研专业课课件] 赫尔《期货、期权及其他衍生产品》课件第10章股票期权的性质

图10-1 股票价格、执行价格、期限变化对期权价格的影响
图10-2 股票波动率、无风险利率的变化对期权价格的影响
10．1．3
波动率
随着波动率的增加，看涨期权及看跌期权价格都会增加。如图10-2a和图10-2b所示。 10．1．4 无风险利率无风险利率增加,看涨期权价格会增加，看跌期权价格会降低。如图10-2c和图1O-2d所示。
例如，如果股票价格是42美元，套利者的盈利
为42-38.96=3.04美元。
考虑以下两个交易组合：组合C：一个欧式看跌期权加上一股股票；组合D：在T时刻收益为K的零息债券。在T时组合C的价值为max（ST，K）组合D的价值为K。
因此在T时刻组合C的价值总是不低于组合D的价值。在无套利条件下，
本节将考虑以上某因素发生变化，而其他因
素保持不变的条件下，对于期权价格的影响。表 10-1 对此进行了总结。
表10-1
一个变量增加而其他变量保持不变
时对于股票期权价格的影响
注：+代表变量增加，期权价格增加；-代表变量增加，期权价格减少；?代表变量增加，期权价格变化不定。
图10-1和图10-2显示了期权价格与以上前5种因素之间的关系，图中采用的参数为：S0=50， K=50，r=5％（每年），σ=20％（每年），r=1 年，并且假定股票无股息。此时看涨期权价格为 7.116，看跌期权价格为4.677。注：S0=50，K=50，r=5％，σ=30％，T=1。
对于一个看涨期权而言，最差的情况是期权
到期时价值为0。因此，期权价值不能为负值，即 c≥0。因此
c max(S0 -Ke rT ,0) （10-4）
例10-2：考虑一个无股息股票的美式看涨期权，假定股票价格为51美元，期权执行价格为50 美元，期权期限为6个月，无风险利率为每年 12％。S0=51美元，K=50美元，T=0.5年，

[考研专业课课件] 赫尔《期货、期权及其他衍生产品》课件第24章信用衍生产品

CD0中，首先需要阐明一个债券组合，然后债券组
合的资金流被一种约定的方式分配到若干类投资者。
24．1
信用违约互换
信用违约互换（credit default swap， CDS）：信用违约互换（保险）的买入方在信用事件发生时有权利将违约公司债券以债券面值的价格卖给信用违约互换的卖出方，CDS的买入方向卖出方定期付款，直到CDS结束或信用事件发生，该合约给某一特定公司的违约风险提供了保险。
每年90个基点违约保护买入方违约保护卖出方
参考实体违约时的支付
图24-1 信用违约互换（CDS）
当没有信用事件发生时，CDS的买入方无任何
收益，并且每个季度有固定支付 0.00225×100 000 000=225 000美元。当有信用事件发生时，卖出方需要向买入方支付赔偿。如果合约指定的交割方式为，买入方有权以1 亿美元的价格向卖出方出售面值为1亿美元、由参
这一次付款之后，买入方不再需要支付任何其他
费用。
相关知识：
CDS的互换溢价（CDS spread）：为了买入信用保护，买入方所付按名义本金百分比计算的数
量。
付款频率：通常为每季度一次，付款时间为期尾。合约的到期日：一般是以下标准日期：3月20 日、6月20日、9月20日和12月20日。违约事件：通常为应当付款时未能支付、债务重组或破产。在北美的合约中，债务重组一般不算违约事件，尤其是在公司债务有很高收益率的情形下。
时间（年ቤተ መጻሕፍቲ ባይዱ 1 2 3
无条件违约概率与生存概率
违约概率 0.0200 0.0196 0.0192 生存概率 0.9800 0.9604 0.9412
4
5
0.0188

赫尔《期权期货及其他衍生产品》第3章(第八版)

15
为什么要对冲股权组合？
• 如果想暂时离开市场一会儿, 那么对冲可以避免出售资产和购买组合的成本。
• 假设你投资组合的β均值为1.0。你认为你的投资组合挑选较为完美, 在任何市场情形下都能战胜市场。对冲可以确保你的收益为无风险收益与投资组合超额收益（相对于市场组合）之和。
期权、期货及其他衍生产品（第八版） Copyright © John C.Hull 2012
• 如果在对冲上有损失、在标的资产上有收益, 如何解释这种情形较为困难。
期权、期货及其他衍生产品（第八版） Copyright © John C.Hull 2012
4
基差风险
• 基差=被对冲风险的即期价格-用于对冲的期货合约的价格 • 对冲被平仓时基差的不确定, 导致了基差风险的产生。
期权、期货及其他衍生产品（第八版） Copyright © • 选择与对冲的到期日最近, 但仍长于对冲到期日的交割月份； • 当被对冲的资产与期货的标的资产不吻合时, 选择期货价格与被对冲
资产的价格相关性最高的期货合约。这就是交叉对冲。
期权、期货及其他衍生产品（第八版） Copyright © John C.Hull 2012
8
最小方差对冲比率的计算(p41)
2
拥护对冲的观点
• 公司应该集中精力发展自身的主要业务, 采取措施将由利率、汇率和其他市场变量所引起的风险降至最低。
期权、期货及其他衍生产品（第八版） Copyright © John C.Hull 2012
3
反对对冲的观点
• 股东通常持有充分分散的投资组合, 他们可以自己对冲面临的风险；
• 当竞争对手选择不对冲风险时, 这会导致对冲风险的上升；
6

期权期货及其衍生产品约翰赫尔官方课件

The value of the portfolio, , is given by ƒ ƒ S S The change in its value in time Dt is given by ƒ D Dƒ DS S
Options, Futures, and Other Derivatives, 8th Edition, Copyright © John C. Hull 2012
10
Nature of Volatility
Volatility is usually much greater when the market is open (i.e. the asset is trading) than when it is closed For this reason time is usually measured in “trading days” not calendar days when options are valued It is assumed that there are 252 trading days in one year for most assets
Options, Futures, and Other Derivatives, 8th Edition, Copyright © John C. Hull 2012
12
The Concepts Underlying BlackScholes-Merton
The option price and the stock price depend on the same underlying source of uncertainty We can form a portfolio consisting of the stock and the option which eliminates this source of uncertainty The portfolio is instantaneously riskless and must instantaneously earn the risk-free rate This leads to the Black-Scholes-Merton differential equation

期权期货及其衍生产品约翰赫尔官方课件共32页文档

P 360 (100 Y ) n
Y is cash price per $100 P is quoted price
Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
6
Treasury Bond Price Quotes in the U.S
Day Count Convention
Defines:
the period of time to which the interest rate applies The period of time used to calculate accrued interest (relevant when the instrument is bought of sold
Cash price = Quoted price + Accrued Interest
Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
7
Treasury Bond Futures
Pages 132-136
Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
9
Conversion Factor
The conversion factor for a bond is approximately equal to the value of the bond on the assumption that the yield curve is flat at 6% with semiannual compounding

[考研专业课课件] 赫尔《期货、期权及其他衍生产品》课件第33章能源与商品衍生产品

能源产品是最重要也是交易最活跃的商品之一，比如原油、天然气和电能，其价格服从均值回归过程。当一种资源的价格上涨时，其消费量很可能会下降，从而对价格产生下跌的压力；当一种资源的价格下跌时，其消费量很可能会上升, 从而对价格产生上涨的压力。
33．3．1 原油
原油市场是世界上最大的商品市场，全球需求量大约为每天8000万桶。由于比重与含硫量的不同，原油分成许多等级。对原油定价的两种重要基准是布兰特原油（Brent crude oil，来自北海）和得州轻油（West Texas Intermediate， WTI）。
格如下，利用期货价格来估计活牛价格在风险中性世界里的增长率。（单位：美分/磅）
气候是决定大多数农产品的关键因素。农作物商品价格的波动率在收获季节之前往往是最高的，而当产量确定后，波动率就会下降。在作物生长季节，随气候的变化，农产品价格所服从的过程往往显示跳跃性。
33．2 金属
金属，包括黄金、白银、铂金、钯、铜、锡、钎、锌、镍和铝。 1．金属的价格不受季节与气候的影响。 2．储存水平、货币汇率波动、不同生产过程中被使用的趋势、所发现这种金属的新产源、勘探技术、开采方法、地缘政治、企业联合以及环保政策等都会对金属价格有影响。
《期权、期货及其他衍生产品》
第33章能源与商品衍生产品
1．介绍商品衍生产品；
2．考虑气候与保险衍生产品。
33．1 农产品
1．农产品的价格是由市场供需决定的。美国农业部定期公布贮备与生产的状况。像棉花与小麦这样的农产品，库存-使用比例（ stocks-to-use ratio），即年终库存量与该年度使用量的比例。一般情况下，这个比例是在20％～40％之间，其对价格波动率有很大影响：如果关于某个商品的这项比例较低，那么商品价格对

期权期货及其衍生产品约翰赫尔官方课件

[考研专业课课件] 赫尔《期货、期权及其他衍生产品》 课件 第34章 实物期权

期权期货及其衍生产品约翰赫尔官方课件97522共21页

期权期货及其衍生产品约翰赫尔官方课件共29页

[考研专业课课件] 赫尔《期货、期权及其他衍生产品》 课件 第28章 利率衍生产品：标准市场模型

期权期货及其衍生产品,约翰赫尔官方课件

期权期货及其衍生产品约翰赫尔官方课件-PPT精选文档

期权期货及其衍生产品约翰赫尔官方课件97522PPT精品文档21页

期货期权及其衍生品配套课件(全34章)Ch03.ppt

赫尔《期权期货及其他衍生产品》第1章(第八版)讲述

期权、期货及其他衍生产品第9版-赫尔】Ch(9)幻灯片PPT

[考研专业课课件] 赫尔《期货、期权及其他衍生产品》 课件 第1章 导 言

期权期货及其衍生产品约翰赫尔官方课件

期权期货及其衍生产品约翰赫尔官方课件

[考研专业课课件] 赫尔《期货、期权及其他衍生产品》 课件 第10章 股票期权的性质

[考研专业课课件] 赫尔《期货、期权及其他衍生产品》 课件 第24章 信用衍生产品

赫尔《期权期货及其他衍生产品》第3章(第八版)

期权期货及其衍生产品约翰赫尔官方课件

期权期货及其衍生产品约翰赫尔官方课件共32页文档

[考研专业课课件] 赫尔《期货、期权及其他衍生产品》 课件 第33章 能源与商品衍生产品

[考研专业课课件] 赫尔《期货、期权及其他衍生产品》课件第34章实物期权

[考研专业课课件] 赫尔《期货、期权及其他衍生产品》课件第28章利率衍生产品：标准市场模型

[考研专业课课件] 赫尔《期货、期权及其他衍生产品》课件第1章导言

[考研专业课课件] 赫尔《期货、期权及其他衍生产品》课件第10章股票期权的性质

[考研专业课课件] 赫尔《期货、期权及其他衍生产品》课件第24章信用衍生产品

[考研专业课课件] 赫尔《期货、期权及其他衍生产品》课件第33章能源与商品衍生产品