MATLAB数学实验练习题
MATLAB全部实验及答案

MATLAB全部实验及答案实验一、MATLAB基本操作实验内容及步骤4、有关向量、矩阵或数组的一些运算(1)设A=15;B=20;求C=A+B与c=a+b?(2)设A=[1 2 3;4 5 6;7 8 9],B=[9 8 7;6 5 4;3 2 1];求A*B与A.*B?A*B就是线代里面的矩阵相乘 A.*B是对应位置的元素相乘(3)设a=10,b=20;求i=a/b=0.5与j=a\b=2?(4)设a=[1 -2 3;4 5 -4;5 -6 7]请设计出程序,分别找出小于0的矩阵元素及其位置(单下标、全下标的形式),并将其单下标转换成全下标。
clear,clca=[1 -2 3;4 5 -4;5 -6 7];[x,y]=find(a<0);c=[];for i=1:length(x)c(i,1)=a(x(i),y(i));c(i,2)=x(i);c(i,3)=y(i);c(i,4)=(y(i)-1)*size(a,2)+x(i);endc(5)在MATLAB命令行窗口运行A=[1,2;3,4]+i*[5,6;7,8];看结果如何?如果改成运行A=[1,2;3,4]+i[5,6;7,8],结果又如何?前面那个是虚数矩阵,后面那个出错(6)请写出完成下列计算的指令:a=[1 2 3;3 4 2;5 2 3],求a^2=?,a.^2=?a^2= 22 16 1625 26 2326 24 28a.^2=1 4 99 16 425 4 9(7)有一段指令如下,请思考并说明运行结果及其原因clearX=[1 2;8 9;3 6];X( : ) 转化为列向量(8)使用三元组方法,创建下列稀疏矩阵2 0 8 00 0 0 10 4 0 06 0 0 0方法一:clear,clcdata=[2 8 1 4 6];ir=[1 1 2 3 4 ];jc=[1 3 4 2 1];s=sparse(ir,jc,data,4,4);full(s)方法二:不用三元组法clear,clca=zeros(4,4);a(1,[1,3])=[2,8];a(2,4)=1;a(3,2)=4;a(4,1)=6;a(9) 写出下列指令的运行结果>> A = [ 1 2 3 ]; B = [ 4 5 6 ];>> C = 3.^A>> D = A.^B5、 已知⎪⎭⎫⎝⎛+⋅=-334sin 234πt e y t 若需要计算t ∈[-1,1],取间隔为0.01,试计算出相对应的y 值。
matlab数学实验练习题

Matlab 数学实验实验一 插值与拟合实验内容:预备知识:编制计算拉格朗日插值的M 文件。
1. 选择一些函数,在n 个节点上(n 不要太大,如5 ~ 11)用拉格朗日、分段线性、三次样条三种插值方法,计算m 个插值点的函数值(m 要适中,如50~100)。
通过数值和图形输出,将三种插值结果与精确值进行比较。
适当增加n ,再做比较,由此作初步分析。
下列函数任选一种。
(1)、 ;20,sin π≤≤=x x y (2)、;11,)1(2/12≤≤--=x x y (3)、;22,c o s10≤≤-=x x y(4)、22),exp(2≤≤--=x x y2.用电压V=10伏的电池给电容器充电,电容器上t 时刻的电压为)(0)()(t eV V V t v ---=,其中0V 是电容器的初始电压,τ是充电常数。
试由下面一组t ,V 数据确定0V 和τ。
实验二 常微分方程数值解试验实验目的:1. 用MATLAB 软件求解微分方程,掌握Euler 方法和龙格-库塔方法;2. 掌握用微分方程模型解决简化的实际问题。
实验内容:实验三地图问题1.下图是一个国家的地图,为了计算出它的国土面积,首先对地图作如下测量:以由西向东方向为x轴,由南到北方向为y轴,选择方便的原点,并将从最西边界点到最东边界点在x轴上的区间适当地划分为若干段,在每个分点的y方向测出南边界点和北边界点的y坐标y1和y2,这样就得到了表中的数据(单位mm)。
根据地图的比例我们知道18mm相当于40km,试由测量数据计算该国土的近似面积,并与它的精确值41288km2比较。
实验四狼追兔问题狼猎兔问题是欧洲文艺复兴时代的著名人物达.芬奇提出的一个数学问题。
当一个兔子正在它的洞穴南面60码处觅食时,一只恶狼出现在兔子正东的100码处。
当两只动物同时发现对方以后,兔子奔向自己的洞穴,狼以快于兔子一倍的速度紧追兔子不放。
狼在追赶过程中所形成的轨迹就是追击曲线。
数学实验matlab练习题

2015-2016数学实验练习题一、选择题1.清除Matlab工作空间(wordspace)变量的命令是(B )A. clcB. clearC. clfD.delete2. 清除当前屏幕上显示的所有内容,但不清除工作空间中的数据的命令是( A )A. clcB. clearC. clfD.delete3. 用来清除图形的命令( C )A. clcB. clearC. clfD.delete4. 在MATLAB程序中,使命令行不显示运算结果的符号是( A )A. ;B. %C. #D. &5. 在MATLAB程序中,可以将某行表示为注释行的符号是( B )A. ;B. %C. #D. &6.在循环结构中跳出循环,执行循环后面代码的命令为 ( B )A. returnB. breakC. continueD. Keyboard7.在循环结构中跳出循环,但继续下次循环的命令为( C )A. returnB. breakC. continueD. Keyboard8. MATLAB中用于声明全局变量的关键字是( C )A. infB. symsC. globalD. function9. 用户可以通过下面哪项获得指令的使用说明( A )A. helpB. loadC. demoD. lookfor10.在MATLAB命令窗口中键入命令S=zoros(3);可生成一个三行三列的零矩阵,如果省略了变量名S,MATLAB表现计算结果将用下面的哪一变量名做缺省变量名( A )A. ans;B. pi;C. NaN;D. Eps.11. 9/0的结果是( B )A. NAN;B. Inf;C. eps;D. 012.在MATLAB中程序或语句的执行结果都可以用不同格式显示,将数据结果显示为分数形式,用下面哪一条命令语句( D )A. format long;B. format long e;C. format bank;D. fromat rat13. 下列MATLAB命令中是构造1行3列的(-1,1)均匀分布随机矩阵的命令的是(D)A. randn(1,3);B. rand(1,3);C. ones(3);D. 以上都不对14. 产生四维元素都为1矩阵的语句为( A )A. ones(4)B. eye(4)C. zeros(4)D. rand(4)15. 用round 函数对数组[2.48 6.39 3.93 8.52]取整,结果为 ( C )A. [2 6 3 8]B. [2 6 4 8]C. [2 6 4 9]D. [3 7 4 9]16. y=dsolve(‘Dy=1/(1+x^2)-2*y^2’,’y(0)=0’,’x ’); ezplot(y)的功能是( A )A. 求微分方程特解并绘图;B. 解代数方程;C. 求定积分;D.求微分方程通解.17. MATLAB 命令roots([1,0,0,-1])的功能是 ( D )A. 产生向量[1,0,0,1];B. 求方程310x +=的根;C. 求多项式31x -的值;D. 求方程310x -=的根。
MATLAB概率习题

数学实验(概率论)题目一.用MATLAB 计算随机变量的分布1.用MA TLAB 计算二项分布在一级品率为0.2的大批产品中,随机地抽取20个产品,求其中有2个一级品的概率。
1. 用MA TLAB 计算泊松分布用MATLAB 计算:保险公司售出某种寿险保单2500份.已知此项寿险每单需交保费120元,当被保人一年内死亡时,其家属可以从保险公司获得2万元的赔偿(即保额为2万元).若此类被保人一年内死亡的概率0.002,试求:(1)保险公司的此项寿险亏损的概率;(2)保险公司从此项寿险获利不少于10万元的概率; (3)获利不少于20万元的概率. 3.用MA TLAB 计算均匀分布乘客到车站候车时间ξ()0,6U ,计算()13P ξ<≤。
4.用MA TLAB 计算指数分布用MA TLAB 计算:某元件寿命ξ服从参数为λ(λ=11000-)的指数分布.3个这样的元件使用1000小时后,都没有损坏的概率是多少? 5。
用MATLAB 计算正态分布 某厂生产一种设备,其平均寿命为10年,标准差为2年.如该设备的寿命服从正态分布,求寿命不低于9年的设备占整批设备的比例? 二.用MATLAB 计算随机变量的期望和方差 1.用MA TLAB 计算数学期望(1)用MATLAB 计算离散型随机变量的期望 1)。
一批产品中有一、二、三等品、等外品及废品5种,相应的概率分别为0.7、0.1、0.1、0.06及0.04,若其产值分别为6元、5.4元、5元、4元及0元.求产值的平均值 2)。
已知随机变量X 的分布列如下:{}kk X p 21== ,,2,1n k =计算.EX (2)用MATLAB 计算连续型随机变量的数学期望假定国际市场上对我国某种商品的年需求量是一个随机变量ξ(单位:吨),服从区间[],a b 上的均匀分布,其概率密度为: 1()0a x bx b aϕ⎧≤≤⎪=-⎨⎪⎩其它计算我国该种商品在国际市场上年销售量的期望.ξE .(3)用MATLAB 计算随机变量函数的数学期望假定国际市场每年对我国某种商品的需求量是随机变量X (单位:吨),服从[20,40]上的均匀分布,已知该商品每售出1吨,可获利3万美元,若销售不出去,则每吨要损失1万美元,如何组织货源,才可使收益最大? 2. 用MA TLAB 计算方差(1)利用MATLAB 计算:设有甲、乙两种股票,今年的价格都是10元,一年后它们的试比较购买这两种股票时的投资风险.。
MATLAB数学实验答案(全)

MATLAB数学实验答案(全)第⼀次练习教学要求:熟练掌握Matlab 软件的基本命令和操作,会作⼆维、三维⼏何图形,能够⽤Matlab 软件解决微积分、线性代数与解析⼏何中的计算问题。
补充命令vpa(x,n) 显⽰x 的n 位有效数字,教材102页fplot(‘f(x)’,[a,b]) 函数作图命令,画出f(x)在区间[a,b]上的图形在下⾯的题⽬中m 为你的学号的后3位(1-9班)或4位(10班以上) 1.1 计算30sin limx mx mx x →-与3sin lim x mx mxx →∞-syms xlimit((902*x-sin(902*x))/x^3) ans =366935404/3limit((902*x-sin(902*x))/x^3,inf)//inf 的意思 ans = 0 1.2 cos1000xmxy e =,求''y syms xdiff(exp(x)*cos(902*x/1000),2)//diff 及其后的2的意思 ans =(46599*cos((451*x)/500)*exp(x))/250000 - (451*sin((451*x)/500)*exp(x))/250 1.3 计算221100x y edxdy +??dblquad(@(x,y) exp(x.^2+y.^2),0,1,0,1)//双重积分 ans = 2.13941.4 计算4224x dx m x +? syms xint(x^4/(902^2+4*x^2))//不定积分 ans =(91733851*atan(x/451))/4 - (203401*x)/4 + x^3/12 1.5 (10)cos ,x y e mx y =求//⾼阶导数syms xdiff(exp(x)*cos(902*x),10) ans =-356485076957717053044344387763*cos(902*x)*exp(x)-3952323024277642494822005884*sin(902*x)*exp(x)1.6 0x =的泰勒展式(最⾼次幂为4).syms xtaylor(sqrt(902/1000+x),5,x)//泰勒展式 ans =-(9765625*451^(1/2)*500^(1/2)*x^4)/82743933602 +(15625*451^(1/2)*500^(1/2)*x^3)/91733851-(125*451^(1/2)*500^(1/2)*x^2)/406802 + (451^(1/2)*500^(1/2)*x)/902 +(451^(1/2)*500^(1/2))/500 1.7 Fibonacci 数列{}n x 的定义是121,1x x ==12,(3,4,)n n n x x x n --=+=⽤循环语句编程给出该数列的前20项(要求将结果⽤向量的形式给出)。
数学实验(matlab版)过程考试试卷及答案完整版

试绘出三种产品产量与季度的三维垂直方向条形图(分组式). >> x=[8,8,9;11,7,8;12,6,9;10,6,10]; bar3(x,'group') 图形如下:
2/2
第一题:编程计算下面问题, x 值由键≥ 1 y = x 2 , −1 ≤ x < 1 2 x − 1, x < −1
>> x=input('输入 x:'); if x>=1 y=x^2+1; end if x<-1 y=x^2-1; end if x>=-1&x<1 y=x^2; end y 输入 x:5 y= 26 第二题:某人做一种材料的伸缩实验,t 为温度(℃),L 为长度(mm),实验数据见下表 t 20 25 30 35 40 L 81 82.3 84 86.8 89
f = x 4 − xy + y 2 ,求
>> syms x y
∂f ∂ 3 f , ∂x ∂y 3
1/1
f=x^4-x*y+y^2; dx=diff(f,x,1) dy3=diff(f,y,3) dx = 4*x^3-y dy3 = 0 第四题:某厂生产三种产品,某年四季度的产量如下 A 产品产量 笫一季度 笫二季度 笫三季度 笫四季度 8 11 12 10 B 产品产量 8 7 6 6 C 产品产量 9 8 9 10
用二阶拟合法,求 L 与 t 的表达式.要求:1.编程;2.写出 L 与 t 的关系式. >> t=[20,25,30,35,40]; L=[81,82.3,84,86.5,89]; k=polyfit(t,L,2) k= 0.0091 -0.1446 80.2114 L=0.0091 t^2 —0.1446t+ 80.2114 第三题:求微分与积分(编程)
MATLAB数学实验第二版课后练习题含答案

MATLAB数学实验第二版课后练习题含答案课后练习题MATLAB数学实验第二版的课后练习题如下:第一章课后练习题1.编写MATLAB程序,计算并输出下列公式的结果:y = \\frac{1}{\\sqrt{2\\pi\\sigma^2}} e^{-\\frac{(x-\\mu)^2}{2\\sigma^2}}其中,x, $\\mu$, $\\sigma$ 分别由用户输入。
要求输出结果精确至小数点后两位。
答案如下:x=input('请输入 x 的值:');mu=input('请输入 mu 的值:');sigma=input('请输入 sigma 的值:');y=1/sqrt(2*pi*sigma^2) *exp(-(x-mu)^2/ (2*sigma^2));fprintf('y = %.2f\', y);2.编写MATLAB程序,求解下列方程的解:4x + y = 11\\\\x + 2y = 7答案如下:A= [4,1;1,2];B= [11;7];X=inv(A) *B;fprintf('x = %.2f, y = %.2f\', X(1), X(2));第二章课后练习题1.编写MATLAB程序,计算下列多项式的值:P(x) = x^4 - 2x^3 + 3x^2 - x + 1其中,x 由用户输入。
要求输出结果精确至小数点后两位。
答案如下:x=input('请输入 x 的值:');y=x^4-2*x^3+3*x^2-x+1;fprintf('P(%.2f) = %.2f\', x, y);2.编写MATLAB程序,绘制下列函数的图像:f(x) = \\begin{cases} x + 1, & x < 0 \\\\ x^2, & 0 \\leq x < 1 \\\\ 2x - 1, & x \\geq 1 \\end{cases}答案如下:x=-2:0.01:2;y1=x+1;y2=x.^2.* ((x>=0) & (x<1));y3=2*x-1;plot(x,y1,x,y2,x,y3);legend('y1 = x + 1','y2 = x^2','y3 = 2x - 1');总结本文提供了《MATLAB数学实验第二版》的部分课后练习题及其答案。
MATLAB数学实验100例题解(修订版)
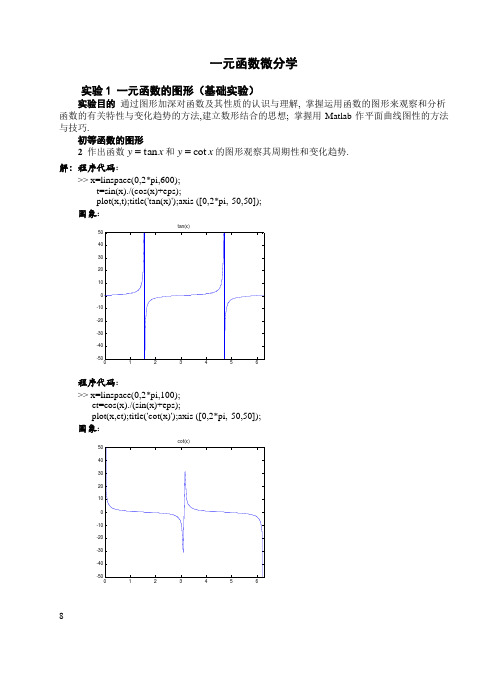
一元函数微分学实验1 一元函数的图形(基础实验)实验目的 通过图形加深对函数及其性质的认识与理解, 掌握运用函数的图形来观察和分析 函数的有关特性与变化趋势的方法,建立数形结合的思想; 掌握用Matlab 作平面曲线图性的方法与技巧.初等函数的图形2 作出函数x y tan =和x y cot =的图形观察其周期性和变化趋势. 解:程序代码:>> x=linspace(0,2*pi,600); t=sin(x)./(cos(x)+eps);plot(x,t);title('tan(x)');axis ([0,2*pi,-50,50]); 图象:程序代码:>> x=linspace(0,2*pi,100); ct=cos(x)./(sin(x)+eps);plot(x,ct);title('cot(x)');axis ([0,2*pi,-50,50]); 图象:4在区间]1,1[-画出函数xy 1sin =的图形. 解:程序代码:>> x=linspace(-1,1,10000);y=sin(1./x); plot(x,y);axis([-1,1,-2,2]) 图象:二维参数方程作图6画出参数方程⎩⎨⎧==t t t y tt t x 3cos sin )(5cos cos )(的图形:解:程序代码:>> t=linspace(0,2*pi,100);plot(cos(t).*cos(5*t),sin(t).*cos(3*t)); 图象:极坐标方程作图8 作出极坐标方程为10/t e r =的对数螺线的图形. 解:程序代码:>> t=0:0.01:2*pi; r=exp(t/10);polar(log(t+eps),log(r+eps)); 图象:90270分段函数作图10 作出符号函数x y sgn =的图形. 解:程序代码:>> x=linspace(-100,100,10000); y=sign(x); plot(x,y);axis([-100 100 -2 2]);函数性质的研究12研究函数)3(log 3)(35x e x x f x -++=在区间]2,2[-上图形的特征. 解:程序代码:>> x=linspace(-2,2,10000);y=x.^5+3*exp(x)+log(3-x)/log(3); plot(x,y); 图象:实验2 极限与连续(基础实验)实验目的 通过计算与作图, 从直观上揭示极限的本质,加深对极限概念的理解. 掌握用 Matlab 画散点图, 以及计算极限的方法. 深入理解函数连续的概念,熟悉几种间断点的图形 特征,理解闭区间上连续函数的几个重要性质.作散点图14分别画出坐标为)10,,2,1(),4,(),,(3222 =+i i i i i i 的散点图, 并画出折线图. 解:散点图程序代码: >> i=1:10; plot(i,i.^2,'.')或:>> x=1:10;y=x.^2;for i=1:10;plot(x(i),y(i),'r')hold onend折线图程序代码:>> i=1:10;plot(i,i.^2,'-x')程序代码:>> i=1:10;plot(i.^2,4*(i.^2)+i.^3,'.')>> i=1:10;plot(i.^2,4*(i.^2)+i.^3,'-x')数列极限的概念16通过动画观察当∞→n 时数列21n a n =的变化趋势.解:程序代码: >> n=1:100; an=(n.^2); n=1:100; an=1./(n.^2); n=1:100; an=1./(n.^2); for i=1:100plot(n(1:i),an(1:i)),axis([0,100,0,1])pause(0.1) %在这个for 循环内每画一个点就暂停0.1s 因此有动画效果 end 图象:函数的极限18在区间]4,4[-上作出函数xx xx x f --=339)(的图形, 并研究 )(lim x f x ∞→ 和 ).(lim 1x f x →解:作出函数x x x x x f --=339)(在区间]4,4[-上的图形 >> x=-4:0.01:4;y=(x.^3-9*x)./(x.^3-x+eps); plot(x,y)从图上看,()f x 在x →1与x →∞时极限为0两个重要极限 20计算极限⎪⎭⎫⎝⎛+→x x x x x sin 11sin lim )1(0 x x e x 2lim )2(+∞→30sin tan lim )3(xx x x -→ x x x 0lim )4(+→ x xx ln cot ln lim )5(0+→ x x x ln lim )6(20+→ xx x x x x sin cos sin lim)7(20-→ 125523lim)8(323+++-∞→x x x x x x x x e e x x x sin 2lim )9(0----→ xx x x cos 110sin lim )10(-→⎪⎭⎫ ⎝⎛ 解:(1)>> limit(x*sin(1/x)+1/x*sin(x))ans =1(2) >> limit(x^2/exp(x),inf) ans = 0(3) >> limit((tan(x)-sin(8))/x^3) ans =NaN(4) >> limit(x^x,x,0,'right') ans =1(5) >> limit(log(cot(x))/log(x),x,0,'right') ans =-1(6) >> limit(x^2*log(x),x,0,'right') ans =016(7) >> limit((sin(x)-x.*cos(x))./(x.^2.*sin(x)),x,0) ans =1/3(8) >> limit((3*x.^3-2*x.^2+5)/(5*x.^3+2*+1),x,inf) ans =3/5(9) >> limit((exp(x)-exp(-x)-2*x)./(x-sin(x))) ans =2(10) >> limit((sin(x)/x).^(1/(1-cos(x)))) ans =exp(-1/3)实验3 导数(基础实验)实验目的 深入理解导数与微分的概念, 导数的几何意义. 掌握用Matlab 求导数与高 阶导数的方法. 深入理解和掌握求隐函数的导数, 以及求由参数方程定义的函数的导数的方法. 导数概念与导数的几何意义22作函数71232)(23+-+=x x x x f 的图形和在1-=x 处的切线. 解:作函数71232)(23+-+=x x x x f 的图形程序代码: >> syms x;>> y=2*x^3+3*x^2-12*x+7; >> diff(y) ans =6*x^2+6*x-12 >> syms x;y=2*x^3+3*x^2-12*x+7; >> f=diff(y) f =6*x^2+6*x-12 >> x=-1;f1=6*x^2+6*x-12 f1 = -12>> f2=2*x^3+3*x^2-12*x+7 f2 = 20>> x=linspace(-10,10,1000);y1=2*x.^3+3*x.^2-12*x+7; y2=-12*(x+1)+20; plot(x,y1,'r',x,y2,'g')求函数的导数与微分24求函数bx ax x f cos sin )(=的一阶导数. 并求.1⎪⎭⎫⎝⎛+'b a f解:求函数bx ax x f cos sin )(=的一阶导数程序代码: >> syms a b x y;y= sin(a*x)*cos(b*x); D1=diff(y,x,1) 答案:D1 =cos(a*x)*a*cos(b*x)-sin(a*x)*sin(b*x)*b求.1⎪⎭⎫ ⎝⎛+'b a f程序代码:>> x=1/(a+b); %直接输入表达式直接带入 >> cos(a*x)*a*cos(b*x)-sin(a*x)*sin(b*x)*b 答案:ans =cos(a/(a+b))*a*cos(b/(a+b))-sin(a/(a+b))*sin(b/(a+b))*b 拉格朗日中值定理26对函数),2)(1()(--=x x x x f 观察罗尔定理的几何意义. (1) 画出)(x f y =与)(x f '的图形, 并求出1x 与.2x 解:程序代码:>> syms x;f=x*(x-1)*(x-2); f1=diff(f) f1 =(x-1)*(x-2)+x*(x-2)+x*(x-1) >> solve(f1) ans =1+1/3*3^(1/2) 1-1/3*3^(1/2)>> x=linspace(-10,10,1000); y1=x.*(x-1).*(x-2);y2 =(x-1).*(x-2)+x.*(x-2)+x.*(x-1); plot(x,y1,x,y2)(2)画出)(x f y =及其在点))(,(11x f x 与))(,(22x f x 处的切线.程序代码:>> syms x; >> f=x*(x-1)*(x-2); >> f1=diff(f) f1 =(x-1)*(x-2)+x*(x-2)+x*(x-1) %本题意在讨论罗尔定理首先回忆一下罗尔定理 >> solve(f1) %(a,b )连续;[a,b]可导;f(a)=f(b)=0;存在ƞ ans = %使得f ’(ƞ)=01+1/3*3^(1/2) %程序就要先求出f (x )的零点 1-1/3*3^(1/2) %再画出f ’(a)f ’(b)的切线 >> x=linspace(-3,3,1000); >> y1=x.*(x-1).*(x-2);>> y2 =(x-1).*(x-2)+x.*(x-2)+x.*(x-1); >> plot(x,y1,x,y2) >> hold on>> x=1+1/3*3^(1/2); >> yx1=x*(x-1)*(x-2) yx1 =-0.3849>> x=1-1/3*3^(1/2);>> yx2=x*(x-1)*(x-2) yx2 =0.3849x=linspace(-3,3,1000); yx1 =-0.3849*x.^0; yx2 =0.3849*x.^0; plot(x,yx1,x,yx2)28求下列函数的导数: (1) 31+=x e y ; 解:程序代码:>> syms x y; y=exp((x+1)^3); D1=diff(y,1) 答案:D1 =3*(x+1)^2*exp((x+1)^3)(2) )]42ln[tan(π+=x y ;解:程序代码:>> syms x;y=log(tan(x/2+pi/4)); D1=diff(y,1) 答案:D1 =(1/2+1/2*tan(1/2*x+1/4*pi)^2)/tan(1/2*x+1/4*pi)(3) x x y sin ln cot 212+=;解:程序代码:>> syms x;y=1/2*(cot(x))^2+log(sin(x)); D1=diff(y,1) 答案:D1 =cot(x)*(-1-cot(x)^2)+cos(x)/sin(x) (4) xy 2arctan21=. 解:程序代码:>> syms x;>> y=sqrt(2)*atan(sqrt(2)/x); >> D1=diff(y,1) 答案:D1 =-2/x^2/(1+2/x^2)一元函数积分学与空间图形的画法实验4 一元函数积分学(基础实验)实验目的 掌握用Matlab 计算不定积分与定积分的方法. 通过作图和观察, 深入理解定积分的概念和思想方法. 初步了解定积分的近似计算方法. 理解变上限积分的概念. 提高应用 定积分解决各种问题的能力.不定积分计算30求.)1(532⎰-dx x x解:程序代码:>> syms x y;>> y=x^2*(1-x^3)^5; >> R=int(y,x) 答案:R =-1/18*x^18+1/3*x^15-5/6*x^12+10/9*x^9-5/6*x^6+1/3*x^332求.arctan 2⎰xdx x 解:程序代码:>> syms x y;>> y=x^2*atan(x); >> R=int(y,x) 答案:R =1/3*x^3*atan(x)-1/6*x^2+1/6*log(x^2+1)定积分计算34 求.)(102⎰-dx x x解:程序代码:>> syms x y; >> y=x-x^2;>> R=int(y,x,0,1) 答案: R =1/6变上限积分 36 画出变上限函数⎰x dt t t 02sin 及其导函数的图形.解:程序代码:>> syms x y t; >> y=t*sin(t^2); >> R=int(y,x,0,x) 答案:R =t*sin(t^2)*x 再求导函数 程序代码:>> DR=diff(R,x,1) 答案:DR =t*sin(t^2)实验5 空间图形的画法(基础实验)实验目的 掌握用Matlab 绘制空间曲面和曲线的方法. 熟悉常用空间曲线和空间曲面 的图形特征,通过作图和观察, 提高空间想像能力. 深入理解二次曲面方程及其图形.一般二元函数作图38作出函数2214y x z ++=的图形.解:程序代码:>> x=linspace(-5,5,500);[x,y]=meshgrid( %mesh 和meshgrid 是生成网格的命令 z=4./(1+x.^2+y.^2); %先命令出一个x 向量组,再生二位网格 mesh(x,y,z); %用meshgrid 再用mesh 命令出各个点的高 xlabel('x-axis'),ylabel('y-axis'),zlabel('z-axis');title('function')40作出函数)94cos(22y x z +=的图形. 解:程序代码:>> x=-10:0.1:10;[x,y]=meshgrid(x);z=cos(4*x.^2+9*y.^2); mesh(x,y,z);xlabel('x-axis'),ylabel('y-axis'),zlabel('z-axis');title('function')讨论:坐标轴选取范围不同时,图形差异很大,对本题尤为明显,如右图为坐标轴[-1,1]二次曲面42作出单叶双曲面1941222=-+z y x 的图形.(曲面的参数方程为 ,tan 3,cos sec 2,sin sec u z v u y v u x === (.20,2/2/πππ≤≤<<-v u ))解:程序代码:>> v=0:pi/100:2*pi; >> u=-pi/2:pi/100:pi/2; >> [U,V]=meshgrid(u,v); >> x=sec(U).*sin(V); >> y=2*sec(U).*cos(V); >> z=3*tan(U); >> surf(x,y,z)44 可以证明: 函数xy z =的图形是双曲抛物面. 在区域22,22≤≤-≤≤-y x 上作出它的图形.解:程序代码:>> x=-2:0.01:2;[x,y]=meshgrid(x); >> z=x.*y;>> mesh(x,y,z);46 画出参数曲面]2,001.0[],4,0[)5/2/ln(tan cos sin sin sin cos ∈∈⎪⎩⎪⎨⎧++===v u u v v z vu y v u x π 的图形.解:程序代码:>> v=0.001:0.001:2;>> u=0:pi/100:4*pi;>> [U,V]=meshgrid(u,v); >> x=cos(U).*sin(V); >> y=sin(U).*sin(V);>> z=cos(V)+log(tan(V/2)+U/5); >> mesh(x,y,z);空间曲线48 作出空间曲线)60(2,sin ,cos π≤≤===t t z t t y t t x 的图形. 解:程序代码:>> syms t;ezplot3(t*cos(t),t*sin(t),2*t,[0,6*pi])-1010-100100xx = t cos(t), y = t sin(t), z = 2 tz50绘制参数曲线 ⎪⎪⎩⎪⎪⎨⎧=+==t z t y t x arctan 211cos 2的图形.解:程序代码:>> t=-2*pi:pi/100:2*pi;x=cos(t).*cos(t);y=1./(1+2*t);z=atan(t); plot3(x,y,z);grid;xlabel('x'),ylabel('y'),zlabel('z')xyz多元函数微积分实验6 多元函数微分学(基础实验)实验目的 掌握利用Matlab 计算多元函数偏导数和全微分的方法, 掌握计算二元函数极值和条件极值的方法. 理解和掌握曲面的切平面的作法. 通过作图和观察, 理解二元 函数的性质、方向导数、梯度和等高线的概念.求多元函数的偏导数与全微分52设),(cos )sin(2xy xy z +=求.,,,222yx z x z y z x z ∂∂∂∂∂∂∂∂∂ 解:程序代码:>> syms x y;S=sin(x*y)+(cos(x*y))^2; D1=diff(S,'x',1); D2=diff(S,'y',1); D3=diff(S,'x',2);D4=diff(S,'y',2); %这是错误的! D1,D2,D3,D4答案: D1 = cos(x*y)*y-2*cos(x*y)*sin(x*y)*yD2 = cos(x*y)*x-2*cos(x*y)*sin(x*y)*xD3 =-sin(x*y)*y^2+2*sin(x*y)^2*y^2-2*cos(x*y)^2*y^2 D4 = -sin(x*y)*x^2+2*sin(x*y)^2*x^2-2*cos(x*y)^2*x^2实验7 多元函数积分学(基础实验)实验目的掌握用Matlab 计算二重积分与三重积分的方法; 深入理解曲线积分、曲面积分的 概念和计算方法. 提高应用重积分和曲线、曲面积分解决各种问题的能力.计算重积分54计算,2dxdy xy D⎰⎰ 其中D 为由,,2y x y x ==+ 2=y 所围成的有界区域.解:程序代码:>> syms x y;int(int(x*y^2,x,2-y,sqrt(y)),y,1,2) 答案:ans =193/120 重积分的应用56求旋转抛物面224y x z --=在Oxy 平面上部的面积.S 解:程序代码:>> int(2*pi*r,r,0,2) 答案: ans =4*pi无穷级数与微分方程实验8 无穷级数(基础实验) 实验目的观察无穷级数部分和的变化趋势,进一步理解级数的审敛法以及幂级数部分和对函数的 逼近. 掌握用Matlab 求无穷级数的和, 求幂级数的收敛域, 展开函数为幂级数以及展 开周期函数为傅里叶级数的方法.数项级数58(1) 观察级数∑∞=121n n的部分和序列的变化趋势.解:程序代码:for i=1:100 s=0; for n=1:i s=s+1/n^2; endplot(i,s,'.');hold on; end(2) 观察级数∑∞=11n n 的部分和序列的变化趋势.>> for i=1:100 s=0; for n=1:i s=s+1/n; endplot(i,s,'.'); hold on; end60 求∑∞=++123841n n n 的值. 解:程序代码:>> syms n;score=symsum(1/(4*n^2+8*n+3),1,inf) %这个用法我比较不熟悉,记一下 答案: score =1/6函数的幂级数展开62求x arctan 的5阶泰勒展开式. >> syms x;>> T5=taylor(atan(x),6) 答案:T5 =x-1/3*x^3+1/5*x^5实验9 微分方程(基础实验)实验目的 理解常微分方程解的概念以及积分曲线和方向场的概念,掌握利用 Matlab 求微分方程及方程组解的常用命令和方法.求解微分方程64求微分方程 22x xe xy y -=+'的通解. 解:程序代码:>> y=dsolve('Dy+2*x*y=x*exp(-x^2)','x') %还记得solve 命令吗?格式solve (‘’) 答案:y = %将可以解一元多次方程而且不用写出等号(1/2*x^2+C1)*exp(-x^2) %本例用于解微分方程,需要声明什么是变量66求微分方程x e y y y x 2cos 52=+'-''的通解. 解:程序代码:>> y=dsolve('D2y-2*Dy+5*y=exp(x)*cos(2*x)','x') %二次倒数‘D2y ’ 答案: y =exp(x)*sin(2*x)*C2+exp(x)*cos(2*x)*C1+1/4*exp(x)*sin(2*x)*x68求微分方程组⎪⎪⎩⎪⎨⎧=--=++02y x dtdy ey x dt dx t 在初始条件0,100====t t y x 下的特解.解:程序代码: %你还别说我真不会接微分方程组>> [x,y]=dsolve('Dx+x+2*y-exp(t)','Dy-x-y','x(0)=1','y(0)=0','t') 答案: x = cos(t)y = 1/2*sin(t)-1/2*cos(t)+1/2*exp(t)70求解微分方程,)1(122/5+=+-x x y dx dy 并作出积分曲线. 解:程序代码:>> syms x yy=dsolve('Dy-2*y/(x+1)-(x+1)^(5/2)','x') 答案:y =(2/3*(x+1)^(3/2)+C1)*(x+1)^2 做积分曲线 由>> syms x yx=linspace(-5,5,100); C=input('请输入C 的值:'); y=(2/3*(x+1).^(3/2)+C).*(x+1).^2; plot(x,y)例如对应有: 请输入C 的值:2 请输入C 的值:20矩阵运算与方程组求解实验10 行列式与矩阵实验目的掌握矩阵的输入方法. 掌握利用Matlab 对矩阵进行转置、加、减、数乘、相乘、乘方等运算, 并能求矩阵的逆矩阵和计算方阵的行列式.矩阵A 的转置函数Transpose[A]72 求矩阵⎪⎪⎪⎪⎪⎭⎫⎝⎛411365243271的转置. 解:程序代码:>> A=[1,7,2;3,4,2;5,6,3;1,1,4]; >> Sove=A' 答案:Sove =1 3 5 1 7 4 6 12 234 矩阵线性运算 73设,291724,624543⎪⎪⎭⎫⎝⎛=⎪⎪⎭⎫⎝⎛=B A 求.24,A B B A -+ 解:程序代码:>> A=[3,4,5;4,2,6]; B=[4,2,7;1,9,2]; S1=A+B S2=4*B-2*A 答案:S1 =7 6 12 5 11 8 S2 =10 0 18 -4 32 -474设,148530291724,36242543⎪⎪⎪⎪⎪⎭⎫ ⎝⎛=⎪⎪⎭⎫ ⎝⎛=mb ma 求矩阵ma 与mb 的乘积. 解:程序代码:>> ma=[3,4,5,2;4,2,6,3];>> mb=[4,2,7;1,9,2;0,3,5;8,4,1];>> Sove=ma*mb答案:Sove =32 65 5642 56 65矩阵的乘法运算 75设,101,530291724⎪⎪⎪⎭⎫ ⎝⎛=⎪⎪⎪⎭⎫ ⎝⎛=B A 求AB 与,A B T 并求.3A解:程序代码:>> A=[4 2 7;1 9 2;0 3 5];B=[1;0;1];>> AB=A*BAB =1135>> BTA=B'*ABTA =4 5 12>> A3=A^3A3 =119 660 555141 932 44454 477 260求方阵的逆76 设,5123641033252312⎪⎪⎪⎪⎪⎭⎫⎝⎛=A 求.1-A解:程序代码:>> A=[2,1,3,2;5,2,3,3;0,1,4,6;3,2,1,5];Y=inv(A)答案:Y =-1.7500 1.3125 0.5000 -0.68755.5000 -3.6250 -2.0000 2.37500.5000 -0.1250 0.0000 -0.1250-1.2500 0.6875 0.5000 -0.312577 设,221331317230,5121435133124403⎪⎪⎪⎪⎪⎭⎫⎝⎛=⎪⎪⎪⎪⎪⎭⎫ ⎝⎛=B A 求.1B A -解:程序代码:>> A=[3 0 4 4 ;2 1 3 3 ;1 5 3 4;1 2 1 5]; B=[0 3 2 ;7 1 3;1 3 3 ;1 2 2];Solve=A'*B %这一步和题目要求不符,应是Solve=inv(A)*B答案:Solve =16 16 1714 20 2225 26 2830 37 3978 解方程组⎪⎩⎪⎨⎧-=-+=+-=++.2442,63,723z y x z y x z y x解:程序代码:>> A=[3 2 1;1 -1 3;2 4 -4]; b=[7 6 -2];>> A\b' %也可以用inv (A )*b ’表达答案:ans =1.00001.00002.0000求方阵的行列式79 求行列式 .3351110243152113------=D 解:程序代码:>> A=[3,1,-1,2;-5,1,3,-4;2,0,1,-1;1,-5,3,-3];D=det(A)答案:D =4080求.11111111111122222222d d d d c cc c b bb b a a a a D ++++= 解:程序代码:>> syms a b c d;D=[a^2+1/a^2 a 1/a 1;b^2+1/b^2 b 1/b 1;c^2+1/c^2 c 1/c 1;d^2+1/d^2 d 1/d 1];det(D)答案:ans =-(-c*d^2*b^3+c^2*d*b^3-c^3*d^2*a+c^3*d*a^2*b^4+c*d^2*a^3-c^3*d^2*a*b^4-c^2*d*a^3-c*d^2*b^3*a^4+c^2*d*b^3*a^4+c^3*d^2*b*a^4-c^3*d*b^2*a^4-c^2*d^3*b*a^4+c*d^3*b^2*a^4+c*d ^2*a^3*b^4-c^2*d*a^3*b^4+c^3*d^2*b-c^3*d*b^2-c^2*d^3*b+c*d^3*b^2+c^3*d*a^2+c^2*d^3*a-c *d^3*a^2-b*d^2*a^3+b^2*d*a^3+b^3*d^2*a-b^3*d*a^2-b^2*d^3*a+b*d^3*a^2+b*c^2*a^3-b^2*c*a ^3-b^3*c^2*a+b^3*c*a^2+b^2*c^3*a-b*c^3*a^2+c^2*d^3*a*b^4-c*d^3*a^2*b^4-b*d^2*a^3*c^4+b ^2*d*a^3*c^4+b^3*d^2*a*c^4-b^3*d*a^2*c^4-b^2*d^3*a*c^4+b*d^3*a^2*c^4+b*c^2*a^3*d^4-b^2*c*a^3*d^4-b^3*c^2*a*d^4+b^3*c*a^2*d^4+b^2*c^3*a*d^4-b*c^3*a^2*d^4)/a^2/c^2/d^2/b^281 计算范德蒙行列式.1111145444342413534333231252423222154321x x x x x x x x x x x x x x x x x x x x解:程序代码:>> syms x1 x2 x3 x4 x5;>> A=[1,1,1,1,1;x1,x2,x3,x4,x5;x1^2,x2^2,x3^2,x4^2,x5^2;x1^3,x2^3,x3^3,x4^3,x5^3;x1^4,x2^4,x3^4,x4^4,x5^4];>> DC=det(A);>> DS=simple(DC)答案:DS =(-x5+x4)*(x3-x5)*(x3-x4)*(-x5+x2)*(x2-x4)*(x2-x3)*(-x5+x1)*(x1-x4)*(x1-x3)*(x1-x2)82 设矩阵 ,60975738723965110249746273⎪⎪⎪⎪⎪⎪⎭⎫⎝⎛----=A 求.),(|,|3A A tr A解:程序代码:>> A=[3,7,2,6,-4;7,9,4,2,0;11,5,-6,9,3;2,7,-8,3,7;5,7,9,0,-6];>> D=det(A),T=trace(A),A3=A^3答案:D =11592T =3A3=726 2062 944 294 -3581848 3150 26 1516 2281713 2218 31 1006 4041743 984 -451 1222 384801 2666 477 745 -125向量的内积83 求向量}3,2,1{=u 与}0,1,1{-=v 的内积.解:程序代码:>> u=[1 2 3];v=[1 -1 0];solve=dot(u,v)答案:solve =-184设,001001⎪⎪⎪⎭⎫ ⎝⎛=λλλA 求.10A 一般地?=k A (k 是正整数).解:程序代码:>> syms r;>> A=[r,1,0;0,r,1;0,0,r];>> A^10答案:ans =[ r^10, 10*r^9, 45*r^8][ 0, r^10, 10*r^9][ 0, 0, r^10]85.求⎪⎪⎪⎪⎪⎪⎭⎫ ⎝⎛+++++a a a aa 1111111111111111111111111的逆. 解:程序代码:>> syms aA=[1+a,1,1,1,1;1,1+a,1,1,1;1,1,1+a,1,1;1,1,1,1+a,1;1,1,1,1,1+a];solve=inv(A)答案:solve =[ 1/a*(a+4)/(a+5), -1/a/(a+5), -1/a/(a+5), -1/a/(a+5), -1/a/(a+5)][ -1/a/(a+5), 1/a*(a+4)/(a+5), -1/a/(a+5), -1/a/(a+5), -1/a/(a+5)][ -1/a/(a+5), -1/a/(a+5), 1/a*(a+4)/(a+5), -1/a/(a+5), -1/a/(a+5)][ -1/a/(a+5), -1/a/(a+5), -1/a/(a+5), 1/a*(a+4)/(a+5), -1/a/(a+5)][ -1/a/(a+5), -1/a/(a+5), -1/a/(a+5), -1/a/(a+5), 1/a*(a+4)/(a+5)] 实验11 矩阵的秩与向量组的极大无关组实验目的 学习利用Matlab 求矩阵的秩,作矩阵的初等行变换; 求向量组的秩与极大无关组. 求矩阵的秩86 设,815073*********⎪⎪⎪⎭⎫⎝⎛-------=M 求矩阵M 的秩.解:程序代码:>> M=[3,2,-1,-3,-2;2,-1,3,1,-3;7,0,5,-1,-8];R=rank(M)答案:R=2向量组的秩87求向量组)0,3,0,2(),2,5,4,0(),1,1,2,1(231=--=-=ααα的秩.解:程序代码:>> A=[1,2,-1,1;0,-4,5,-2;2,0,3,0];R=rank(A)答案:R =288向量组)7,5,1,3(),5,4,3,1(),1,1,1,1(),3,2,1,1(4321==-==αααα是否线性相关?解:由>> A=[1 1 2 3;1 -1 1 1;1 3 4 5;3 1 5 7];rank(A)ans = 3即rank(A)=3 小于阶数489向量组)3,1,1(),2,1,3(),7,2,2(321=-==ααα是否线性相关?解:由>> A3=[2,2,7;3,-1,2;1,1,3];R=rank(A3)得 R = 3即rank(A3)=3 等于阶数3故向量组线性无关。
matlab数学实验考试题及答案

matlab数学实验考试题及答案一、选择题(每题2分,共10分)1. MATLAB中用于生成0到1之间均匀分布的随机数的函数是?A. randB. randiC. randnD. randperm答案:A2. 下列哪个命令可以计算矩阵的行列式?A. detB. rankC. eigD. inv答案:A3. MATLAB中用于求解线性方程组的命令是?A. solveB. linsolveC. fsolveD. ode45答案:A4. 在MATLAB中,如何创建一个3x3的单位矩阵?A. eye(3)B. ones(3)C. zeros(3)D. identity(3)答案:A5. MATLAB中用于绘制二维图形的函数是?A. plotB. surfC. meshD. contour答案:A二、填空题(每题3分,共15分)1. MATLAB中,使用________函数可以计算矩阵的迹。
答案:trace2. 若要在MATLAB中创建一个从1到10的向量,可以使用________函数。
答案:1:103. MATLAB中,使用________函数可以计算矩阵的特征值。
答案:eig4. 若要在MATLAB中绘制一个正弦波,可以使用________函数。
答案:sin5. MATLAB中,使用________函数可以计算矩阵的逆。
答案:inv三、简答题(每题10分,共20分)1. 描述MATLAB中如何使用循环结构来计算并打印1到100之间所有奇数的和。
答案:可以使用for循环结构,初始化一个变量sum为0,然后遍历1到100之间的每个数,使用模运算符判断是否为奇数,如果是,则将其加到sum上,最后打印sum的值。
2. 简述MATLAB中如何使用条件语句来检查一个数是否为素数,并打印出所有小于100的素数。
答案:可以使用for循环遍历2到99之间的每个数,对于每个数,使用一个while循环检查它是否有除1和它本身之外的因数,如果没有,则使用if语句判断该数是否为素数,如果是,则打印该数。
数学实验(matlab)样题及参考解答

东华大学高等数学实验试题A考试时间:90分钟(附参考解答)班级 学号 姓名 得分 上机考试说明:1. 开考前可将准备程序拷到硬盘, 开考后不允许用移动盘,也不允许上网;2. 领座考生试卷不同,开卷,可利用自己备用的书和其他资料,但不允许讨论,也不允许借用其他考生的书和资料。
3. 解答(指令行,答案等)全部用笔写在考卷上。
一、 计算题(70分)要求:写出M 函数(如果需要的话)、MATLAB 指令和计算结果。
1. 解线性方程组⎪⎪⎩⎪⎪⎨⎧-=+=+--=-+=-+14235231543421431321x x x x x x x x x x x 并求系数矩阵的行列式。
指令行:A=[5 1 –1 0;1 0 3 –1;-1 –1 0 5;0 0 2 4];b=[1;2;3;-1]; x=A\b,d=det(A) 结果:x 1=1.4, x 2= -5.9, x 3=0.1, x 4= -0.3. 行列式=70.2. 设 f(x,y) = 4 sin (x 3y),求 3,22==∂∂∂y x y x f 。
指令行:syms x y; f=diff(4*sin(x^3*y),x); f=diff(f,y); f=subs(f,x,2); f=subs(f,y,3)结果:1063.63. 求方程 3x 4+4x 3-20x+5 = 0 的所有解。
指令行:roots([3 4 0 –20 5])结果:-1.5003 - 1.5470i, -1.5003 + 1.5470i, 1.4134, 0.25394. 使用两种方法求积分dx e x 210221-⎰π的近似值。
方法一:指令行:syms x; s=int(1/sqrt(2*pi)*exp(-x^2/2),0,1); vpa(s,5)结果:0.34135方法二:指令行:x=0:0.01:1; y=1/sqrt(2*pi)*exp(-x.^2/2);trapz(x,y)结果:0.3413方法三:M 函数ex4fun.mfunction f=ex4fun(x)f=1/sqrt(2*pi)*exp(-x.^2/2);指令行:s=quadl(@ex4fun,0,1)结果:0.34135. 求函数 f(x,y) = 3x 2+10y 2+3xy-3x +2y 在原点附近的一个极小值点和极小值。
matlab数学实验复习题(有答案)

matlab数学实验复习题(有答案)复习题1、写出3个常用的绘图函数命令2、inv (A )表示A 的逆矩阵;3、在命令窗口健入clc4、在命令窗口健入clear 5、在命令窗口健入6、x=-1:0.2:17、det (A )表示计算A 的行列式的值;8、三种插值方法:拉格朗日多项式插值,分段线性插值,三次样条插值。
9、若A=123456789⎡⎤⎢⎥⎢⎥⎢⎥⎣⎦,则fliplr (A )=321654987⎡⎤⎢⎥⎢⎥⎢⎥⎣⎦A-3=210123456--⎡⎤⎢⎥⎢⎥⎢⎥⎣⎦A .^2=149162536496481⎡⎤⎢⎥⎢⎥⎢⎥⎣⎦tril (A )=100450789⎡⎤⎢⎥⎢⎥⎢⎥⎣⎦ triu (A ,-1)=123456089⎡⎤⎢⎥⎢⎥⎢⎥⎣⎦diag (A )=100050009⎡⎤⎢⎥⎢⎥⎢⎥⎣⎦A(:,2),=258A(3,:)=369 10、normcdf (1,1,2)=0.5%正态分布mu=1,sigma=2,x=1处的概率[t,x]=ode45(@f,[a,b],x0),中参数的涵义是@fun 是求解方程的函数M 文件,[a,b]是输入向量即自变量的范围a 为初值,x0为函数的初值,t function 开头;1721、设x )的功能是作出将X 十等分的直方图22、interp1([1,2,3],[3,4,5],2.5)Ans=4.523、建立一阶微分方程组⎩⎨⎧+='-='yx t y y x t x 34)(3)(2的函数M 文件。
(做不出来)二、写出运行结果:1、>>eye(3,4)=1000010000102、>>size([1,2,3])=1;33、设b=round (unifrnd (-5,5,1,4)),则=3 5 2 -5 >>[x,m]=min(b);x=-5;m=4,[x,n]=sort(b)-5 2 3 5 4 3 1 2mean(b)=1.25,median (b )=2.5,range (b )=104、向量b 如上题,则>>any(b),all(b<2),all(b<6)Ans=1 0 15、>>[5 6;7 8]>[7 8;5 6]=00116、若1234B ⎡⎤=⎢⎥⎣⎦,则 7、>>diag(diag(B))=10048、>>[4:-2:1].*[-1,6]=-4 129、>>acos(0.5),atan(1)ans=1.047197551196598ans=0.78539816339744810、>>norm([1,2,3])Ans=3.74165738677394111、>>length([1,3,-1])=312、>>x=0:0.4:2;plot(x,2*x,’k*’)13、>>zeros(3,1);ans=14、>>ones(3)=111111111,vander([2,3,5])=421931255116、>>floor(1:0.3:3)=1 1 1 12 2 218、>>subplot(2,2,1); fplot('sin',[0,2*pi]);subplot(2,2,2);plot([1,2,-1]);>>x=linspace(0,6*pi);subplot(2,2,3);plot3(cos(x),sin(x),x);>>subplot(2,2,4);polar(x,5*sin(4*x/3));19、>>t=linespace(0,2,11)0.0 0.2 0.4 0.6 0.8 1.0 1.2 1.4 1.6 1.8 2.020、>>[a,b]=binostat(15,0.2)a=3 b=2.4>>y1=binopdf(5,10,0.7)=0.1029,y2=binocdf(5,10,0.7)=0.15031 1 1 11 1 1 1>>y=-poissrnd(8,2,4)-16 -10 8 -7-7 -8 -6 -9>>sign(y)-1 -1 -1 -1-1 -1 -1 -135、>>[a1,b1]=binostat(20,0.4) a1=8 b1=4.8 >>[a2,b2]=poisstat(8)ans=8,8>>[a3,b3]=chi2stat(15)ans=[15 30]36、运行M文件:chi2fign=5;a=0.9;xa=chi2inv(a,n);x=0:0.1:15;y=chi2pdf(x,n);plot(x,y,'b');hold on;xf=0:0.1:xa;yf=chi2pdf(xf,n);fill([xf,xa],[yf,0],'g');text(xa*1.01,0.005,num2str(xa));text(2.5,0.05,'alpha=0.9','fontsize',20); text(9,0.09,'X~{\chi}^2(4)','fontsize',16);37、>>t=linspace(0,2*pi);>>polar(t,3*t,’g*’)38、>>quadl(’exp(2*x).*log(3*x)’,1,3)ans =398.635239、x0=0:2*pi/6:2*pi;y0=sin(x0).*cos(x0);x=[linspace(0,2*pi,100)];y=sin(x).*cos(x);y1=spline(x0,y0,x); [x;y;y1]'plot(x,y,'k',x,y1,'b-')注:此处省略100组数据40、>>A=round(unifrnd(0,100,3,3));>>[L,U]=lu(A)L =0.9897 0.4699 1.00000.1649 1.0000 01.0000 0 0U =97.0000 80.0000 92.00000 35.8041 26.82470 0 -89.656841、a=sparse([1 3 3],[2 3 5],[1 2 3],4,5);s=full(a)s =0 1 0 0 00 0 0 0 00 0 2 0 30 0 0 0 0三、编程1、 分别用矩形公式、梯形公式、辛普森公式、Gauss-Lobatto 公式及随机模拟方法计算数值积分/230sin 2x e xdx π⎰,并与符号运算计算的结果进行比较。
matlab与数学实验的考试试题

matlab与数学实验的考试试题一、单项选择题(每题2分,共10分)1. MATLAB的全称是什么?A. Matrix LaboratoryB. Microprocessor Application ToolC. Microsoft Advanced Technology ToolD. Microprocessor Application Technology2. 在MATLAB中,以下哪个命令用于绘制函数f(x)=x^2在闭区间[0,1]上的图像?A. plot(0:1, 0:1)B. plot(0:0.01:1, 0:0.01:1)C. plot(0:1, 0:1:1)D. plot(0:0.01:1, 0.^2)3. 以下哪个MATLAB命令用于求解线性方程组?A. solveB. linsolveC. equationD. linear4. 在MATLAB中,用于生成一个3x3单位矩阵的命令是什么?A. eye(3)B. unit(3)C. identity(3)D. I(3)5. 如果变量x和y在MATLAB中分别表示为x = [1 2 3; 4 5 6] 和 y= [1; 2; 3],那么表达式x * y的结果是什么?A. [5; 15; 29]B. [14; 32; 50]C. [7; 15; 23]D. [3; 6; 9]二、简答题(每题5分,共20分)1. 简述MATLAB在数学实验中的作用和重要性。
2. 解释MATLAB中向量和矩阵的区别,并给出创建它们的基本命令。
3. 在MATLAB中,如何使用for循环生成一个从1到100的奇数向量?4. 描述在MATLAB中使用函数文件的过程,包括如何定义和调用函数。
三、编程题(每题10分,共30分)1. 编写一个MATLAB函数,该函数接受一个向量作为输入,并返回向量中所有元素的和。
```matlabfunction S = sumVector(V)% 请在此处编写代码end```2. 编写一个MATLAB脚本,该脚本生成一个5x5的随机矩阵,并计算其行列式。
Matlab数学规划方法及实验题目

MATLAB数学规划问题(实验题目及答案在最后)一、线性规划线性规划问题是目标函数和约束条件均为线性函数的问题,MATLAB6.0及更高版本解决的线性规划问题的标准形式为:min n R',f∈xxsub.to:b⋅A≤x⋅Aeq=xbeq≤lb≤xub其中f、x、b、beq、lb、ub为向量,A、Aeq为矩阵。
其它形式的线性规划问题都可经过适当变换化为此标准形式。
在MATLAB6.0版中,线性规划问题(Linear Programming)已用函数linprog取代了MATLAB5.x版中的lp函数。
在6.0和7.0中依然可以使用lp 函数,但在更高版本中,就只能使用linprog函数了。
函数linprog调用格式:x=linprog(f,A,b)x=linprog(f,A,b,Aeq,beq)- 1 -- 1 -x=linprog(f,A,b,Aeq,beq,lb,ub) x=linprog(f,A,b,Aeq,beq,lb,ub,x0) x=linprog(f,A,b,Aeq,beq,lb,ub,x0,options) [x,fval]=linprog(…)[x, fval, exitflag]=linprog(…) [x, fval, exitflag, output]=linprog(…)[x, fval, exitflag, output, lambda]=linprog(…) 说明:x=linprog(f, A, b) %求min f ' *x, sub.to b x A ≤⋅线性规划的最优解。
返回值x 为最优解向量。
x=linprog(f, A, b, Aeq, beq) %含有等式约束beq x Aeq =⋅,若没有不等式约束b x A ≤⋅,则令A=[ ],b=[ ]。
x = linprog(f, A, b, Aeq, beq, lb, ub) %指定x 的范围ub x lb ≤≤ x=linprog(f, A, b, Aeq, beq, lb, ub, x0) %设置x0为初值点。
数学实验第二次测验题及参考答案(09级)

数学实验第二次测验题及参考答案(09级)数学实验第二次测验题及参考答案一、写出下列MATLAB指令的运算结果.1. A=[1;2;3]; transpose(A)1 2 31 2 32. A=[1, 2, 3 ; 4, 5, 6 ]; B=A([1 2], [1,3]) , d=size(A)B =1 34 6d =2 33. a=1:3; b=linspace(1,3,3); x=sum(a.*b), y=cross(a, b)x = 14y = 0 0 04. A=[1,2, 3; 4,5,6; 7,8,9]; B=ones(3); C=A-BC =0 1 23 4 56 7 85. v=[1, 2, 3]; A=diag(v); E=eig(A), D=det(A)E=123D =66. x=[1,2,3,4,5]; [mean(x), median(x), range(x),sum(x), prod(x)]3 34 15 1207. x=[2,3,4]; a=cumsum(x) ,b=sort(x)a =2 5 9b =2 3 48.format rat; v=[1, 2, 3]; A=diag(v); inv(A)ans =1 0 00 1/2 00 0 1/39. [m,v]=normstat(1,4) % 求参数为1,4的正态分布的均值与方差m =1, v =16二、写出下列MATLAB指令的实验目的.1. dsolve('x*Dy+y-exp(-x)=0', 'y(1)=2*exp(1)', 'x')求微分方程0=-+'-x e y y x 在初始条件e y x 2|1==下的特解.2. u=[1,2,3],v=[0,3,2], w=[5, 2, 1]; dot(w, cross(u, v))计算向量u, v, w 的混合积.3. A=[1 2 3; 2 2 5; 3 5 1]; b=[1;2;3]; det(A); inv(A)*b利用逆矩阵解线性方程组=++=++=++3532522132321 321321x x x x x x x x x .4. A=[0 0 1; 0 1 1; 1 1 1; 1 0 0]; rref(A)求向量组)1,0,0(1=α,)1,1,0(2=α,)1,1,1(3=α,)0,0,1(4=α的秩.或对矩阵A 做行初等变换。
Matlab实验习题集答案

1:用以上两种形式计算36sin 5e ++π算术运算结果。
>> 5^6+sin(pi)+exp(3)ans =1.5645e+004>> x=5^6+sin(pi)+exp(3)x = 1.5645e+0042:已知矩阵⎥⎦⎤⎢⎣⎡=⎥⎦⎤⎢⎣⎡=2211,2121B A ,对它们做简单的关系与逻辑运算C=(A<B)&(A= =B)>> A=[1 2;1 2];>> B=[1 1;2 2];>> C=(A<B)&(A==B) C =0 00 03:对数7sin 5+=a 用五位定点、十五位定点以及有理数形式表示出来。
>> a=5+sin(7);format short,aa =5.6570>> a=5+sin(7);>> format long,aa =5.6569865987187894:直接输入创建矩阵⎥⎥⎥⎦⎤⎢⎢⎢⎣⎡=98760154321A>> A=[1 2 3;4 15 60;7 8 9]A =1 2 34 15 607 8 95:输入矩阵111111111⎛⎫ ⎪ ⎪ ⎪⎝⎭。
%利用MATLAB 命令直接输入矩阵OneMatrix=ones(3,3,1)OneMatrix =1 1 1 1 1 1 1 1 16:输入矩阵00000 00000⎛⎫ ⎪⎝⎭>> OneMatrix=ones(2,5,1);ZeroMatrix=zeros(size(OneMatrix))ZeroMatrix =0 0 0 0 00 0 0 0 07:生成3阶魔方矩阵。
>> magic(3)ans =8 1 63 5 74 9 28:操作符冒号”:”的应用a)步长为1的等差数列b)步长为2的等差数列c)步长为-2的等差、递减数列>> 0:1:10ans =0 1 2 3 4 5 6 7 8 9 10 >> 0:2:10ans =0 2 4 6 8 10>> 10:(-2):0ans =10 8 6 4 2 09:已知矩阵⎥⎥⎥⎦⎤⎢⎢⎢⎣⎡=162ln973sin56231A,抽取与修改矩阵A的一些元素.a)求矩阵A的第二行第三列元素b)求矩阵A的第四个元素c)取矩阵A的A(2),A(3),A(4)d)取矩阵A的第一行e)取矩阵A的第三列f)把矩阵A的第一行第三列元素赋值给变量g)把矩阵A的第二行第一列元素修改为100>> A=[1 23 56;sin(3) 7 9;log(2) 6 1] >> A(2,3)ans =9>> A(4)ans =23>> A(2),A(3),A(4)ans =0.141120008059867ans =0.693147180559945ans =23>> A(1,:)ans =1 23 56>> A(:,3)ans =5691>> x=A(1,3)x =56>> A(2,1)=100A =1.0000 23.0000 56.0000 100.0000 7.0000 9.00000.6931 6.0000 1.000010:已知矩阵⎥⎦⎤⎢⎣⎡=⎥⎦⎤⎢⎣⎡=851,9631BA,利用A与B生成矩阵13100690C⎛⎫= ⎪⎝⎭,() D A B=,AAAB⎛⎫= ⎪⎝⎭。
数学实验(MATLAB版韩明版)2.1-2.4部分答案

数学实验(MATLAB版韩明版)2.1-2.4部分答案练习2.1画出下列常见曲线的图形(其中a=1,b=2,c=3)1.31xy =的图像:()55≤≤-x编程:>> x=-5:0.1:5; >> y=x.^(1/3); >> plot(x,y) >> grid on>> xlabel('x');ylabel('y') >> legend('y=x.^(1/3)') >> title('y=x.^(1/3)') 图像:-5-4-3-2-101234500.20.40.60.811.21.41.61.8xyy=x.(1/3)y=x.(1/3)2.e x y -=2的图像:()55≤≤-x 编程:>> x=-5:0.1:5; >> y=exp(-x.^2); >> plot(x,y) >> grid on>> xlabel('x');ylabel('y') >> legend('y=e^(-x^2)'); >> title('y=e^(-x^2)') 图像:-5-4-3-2-101234500.10.20.30.40.50.60.70.80.91xyy=e (-x 2)y=e (-x 2)=++=+=axy a y at x yx tt t313,1333222的图像:()55≤≤-x ,a=1编程:>> t=-5:0.1:5;>> x=3*t./(1+t.^2);y=3*t.^2./(1+t.^2); >> plot(x,y) >> grid on>> xlabel('x');ylabel('y')>> title('x=3*t./(1+t.^2);y=3*t.^2./(1+t.^2)') >> legend('x=3*t./(1+t.^2);y=3*t.^2./(1+t.^2)') 图像:-1.5-1-0.500.51 1.500.511.522.53xyx=3*t./(1+t.2);y=3*t.2./(1+t.2)4.?+=+=x a a y a x xyt tt t3223221,1的图像: ()55≤≤-t ,a=1 编程:>> t=-5:0.1:5;>> x=t.^2./(1+t.^2);y=t.^3./(1+t.^2); >> plot(x,y)>> xlabel('x');ylabel('y')>> title('x=t.^2./(1+t.^2);y=t.^3./(1+t.^2)') >> legend('x=t.^2./(1+t.^2);y=t.^3./(1+t.^2)') >> grid on 图像:0.10.20.30.40.50.60.70.80.91-5-4-3-2-1012345xyx=t.2./(1+t.2);y=t.3./(1+t.2)5.()()t b y t t a x cos 1,sin -=-=的图像:pi t pi *2*2≤≤-,a=1,b=2 编程:>> t=-2*pi:0.1:2*pi; >> x=t-sin(t);y=2*(1-cos(t)); >> plot(x,y) >> grid on>> xlabel('x');ylabel('y')>> legend('x=t-sin(t);y=2*(1-cos(t))') >> title('x=t-sin(t);y=2*(1-cos(t))') 图像:-8-6-4-20246800.511.522.533.54xyx=t-sin(t);y=2*(1-cos(t))x=t-sin(t);y=2*(1-cos(t))6.=+==ayx t a y t a x 32323233sincos ,的图像:pi t pi *2*2≤≤-,a=1 编程:>> t=-2*pi:0.1:2*pi; >> x=(cos(t)).^3;y=(sin(t)).^3; >> plot(x,y) >> grid on>> xlabel('x');ylabel('y')>> title('x=(cos(t)).^3;y=(sin(t)).^3') 图像:-1-0.8-0.6-0.4-0.200.20.40.60.81-1-0.8-0.6-0.4-0.200.20.40.60.81xyx=(cos(t)).3;y=(sin(t)).3x=(cos(t)).3;y=(sin(t)).37.ct z t b y t a x ===,sin ,cos 的图像:()pi t pi c b a *2*2,3,2,1≤≤-=== 编程:>> t=-2*pi:0.1:2*pi; >> x=cos(t);y=2*sin(t);z=3*t; >> plot3(x,y ,z)>> xlabel('x');ylabel('y');zlabel('z') >> grid on>> legend('x=cos(t);y=2*sin(t);z=3*t') >> title('x=cos(t);y=2*sin(t);z=3*t') 图像:-101-2-1012-20-101020xx=cos(t);y=2*sin(t);z=3*tyzx=cos(t);y=2*sin(t);z=3*t8.θa r =的图像:()pi a *20,1≤≤=θ编程:>> theta=0.0:0.1:2*pi; >> r=theta; >> polar(theta,r) >> grid on>> legend('r=theta') >> title('r=theta') 图像:24 68302106024090270120300150330180r=theta r=theta9.e a r θ=的图像:()pi a *20,1≤≤=θ编程:>> theta=-2*pi:0.1:2*pi; >> r=exp(theta); >> polar(theta,r) >> grid on >> title('r=exp(theta)') >> legend('r=exp(theta)') 图像:100200 300400 5003021060240902701203001503301800r=exp(theta)r=exp(theta)10.()?-==+yx ayxar 22222222,2cos θ的图像:1=a 编程:>> theta=0:0.1:2*pi;>> r=sqrt(abs(cos(2*theta))); >> polar(theta,r) >> grid on>> title('r=sqrt(abs(cos(2*theta)))'); >> legend('r=sqrt(abs(cos(2*theta)))') 图像:0.20.4 0.60.8 13021060240902701203001503301800r=sqrt(abs(cos(2*theta)))11.()==+xy a yxar 2222*222,2sin θ的图像:a=1编程:>> theta=0:0.1:2*pi;>> r=sqrt(abs(sin(2*theta))); >> polar(theta,r) >> grid on>> title('r=sqrt(abs(sin(2*theta)))') >> legend('r=sqrt(abs(sin(2*theta)))') 图像:0.4 0.60.8 13021060240902701203001503301800r=sqrt(abs(sin(2*theta)))12.)cos 1(θ+=a r 的图像:a=1 编程:>> theta=0:0.1:2*pi; >> r=1+cos(theta); >> polar(theta,r) >> grid on >> legend('r=1+cos(theta)') >> title('r=1+cos(theta)') 图像:0.51 1.52302106024090270120300150330180r=1+cos(theta)r=1+cos(theta)练习2.21.求出下列极限值. (1)nnn n33+∞→;(2)()n n n n ++-+∞→122lim;(3)x x x 2cot lim→;(4)??? ?→x m xx cos lim 0;(5)--→111lim1e xx x ;(6)??-+∞→x x xx 2lim .解:(1)编程:>> syms n >> limit((n^3+3^n)^(1/n),n,inf) ans = 3(2)编程:>> syms n>> limit(sqrt(n+2)-2*sqrt(n+1)+sqrt(n),n,inf) ans =0(3)编程:>> syms x >> limit(x*cot(2*x),x,0) ans = 1/2(4)编程:>> syms x m >> limit((cos(m/x))^x,x,inf) ans =1(5)编程:>> syms x>> limit(1/x-1/(exp(x)-1),x,1) ans = (exp(1)-2)/(exp(1)-1) (6)编程:>> syms x >> limit(sqrt(x^2+x)-x,x,1) ans = 2^(1/2)-1 2.有个客户看中某套⾯积为180m 2,每平⽅⽶7500元的房⼦。
MATLAB程序设计插值实验题目

1、当2,1,1-=x 时,4,3,0)(-=x f ,求x =0时的值。
2、给出x x f ln )(=的数值表用线性插值及二次插值计算54.0ln 的近似值。
3、4()31f x x x =++,已知x =0,1,2处的值,计算x =0.11的近似值。
4.求i =3和5时的值。
5. 给定数据表,采用牛顿插值方法求i =3和5时的值。
6. 已知函数y =在4, 6.25,9x x x ===的近似值。
7. 已知函数y =在4,9,16x x x ===的近似值。
8. 已知函数y =在0,1,4,9x x x x ==== 9. 给定数据表:构造出函数()f x 的差商表,并计算x =0.5时的值.10. 已知函数y =在8,27,64x x x ===的近似值。
11. 已知函数sin()y x =在0,/4,/2x x x ππ===处的函数值,试通过一个二次插值函数求sin(/5)π的近似值。
12. 已知函数cos()y x =在0,/4,/2x x x ππ===处的函数值,试通过一个二次插值函数求cos(/5)π的近似值。
13. 已知函数tan()y x =在0,/4,/3x x x ππ===处的函数值,试通过一个二次插值函数求tan(/5)π的近似值。
14. 已知函数4y x =在1,2,3x x x ===处的函数值,试通过一个二次插值函数求 1.111x =的近似值。
15. 已知函数sin()y x =在0,/4,/2x x x ππ===处函数值,通过一个二次插值函数求1sin(2/5)π-的近似值。
16. 已知函数cos()y x =在0,/4,/2x x x ππ===处函数值,通过一个二次插值函数求1+cos(/5)π的近似值。
17. 已知函数5y x =在1,2,3x x x ===处的函数值,试通过一个二次插值函数求 1.121x =的近似值。
18. 已知函数41/y x =在1,2,3x x x ===处的函数值,试通过一个二次插值函数求 1.211x =的近似值。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
“MATLAB”练习题要求:抄题、写出操作命令、运行结果,并根据要求,贴上运行图。
1、求230x e x -=的所有根。
(先画图后求解)(要求贴图)>> solve('exp(x)-3*x^2',0) > ezplot('exp(x)-3*x^2') >> grid on ans =-2*lambertw(-1/6*3^(1/2)) -2*lambertw(-1,-1/6*3^(1/2)) -2*lambertw(1/6*3^(1/2))2、求下列方程的根。
1) 5510x x ++=a=solve('x^5+5*x+1',0);a=vpa(a,6) a =1.10447+1.05983*i -1.00450+1.06095*i -.199936 -1.00450-1.06095*i 1.10447-1.05983*i2)1sin 02x x -=至 少三个根>> fzero('x*sin(x)-1/2', 3)ans =2.9726>> fzero('x*sin(x)-1/2',-3)ans =-2.9726>> fzero('x*sin(x)-1/2',0)ans =-0.74083)2sin cos 0x x x -= 所有根>> fzero('sin(x)*cos(x)-x^2',0)ans =>> fzero('sin(x)*cos(x)-x^2',0.6)ans =0.70223、求解下列各题: 1)30sin limx x xx->->> sym x;>> limit((x-sin(x))/x^3,x,0) ans = 1/62) (10)cos ,x y e x y =求 >> sym x>> diff(exp(x)*cos(x),10) ans =(-32)*exp(x)*sin(x)3)21/2(17x e dx⎰精确到位有效数字)>> sym x>> vpa((int(exp(x^2),x,0,1/2)),17) ans =0.544987104183622224)42254x dx x +⎰>> sym x;>> int(x^4/(25+x^2),x) ans =125*atan(x/5) - 25*x + x^3/35)求由参数方程arctan x y t⎧⎪=⎨=⎪⎩所确定的函数的一阶导数dy dx 与二阶导数22d y dx 。
>> syms t>> x=log(sqrt(1+t^2));y=atan(t); >> diff(y,t)/diff(x,t) ans = 1/t6)设函数y =f (x )由方程xy +e y = e 所确定,求y ′(x )。
>> syms x y;f=x*y+exp(y)-exp(1); >> -diff(f,x)/diff(f,y) ans =-y/(x + exp(y))7)sin2xe xdx+∞-⎰>> syms x;>> y=exp(-x)*sin(2*x);>> int(y,0,inf)ans =2/58)08x=展开(最高次幂为)>> syms xf=sqrt(1+x);taylor(f,0,9)ans =- (429*x^8)/32768 + (33*x^7)/2048 - (21*x^6)/1024 + (7*x^5)/256 - (5*x^4)/128 + x^3/16 - x^2/8 + x/2 + 19)1sin(3)(2)xy e y=求>> syms x y;>> y=exp(sin(1/x));>> dy=subs(diff(y,3),x,2)dy =-0.582610)求变上限函数xx⎰对变量x的导数。
>> syms a t;>> diff(int(sqrt(a+t),t,x,x^2))Warning: Explicit integral could not be found.ans =2*x*(x^2 + a)^(1/2) - (a + x)^(1/2)4、求点(1,1,4)到直线L :31102x y z --==- 的距离>> M0=[1,1,4];M1=[3,0,1];M0M1=M1-M0; v=[-1,0,2];d=norm(cross(M0M1,v))/norm(v) d =1.09545、已知22()2(),x f x μσ--=分别在下列条件下画出()f x 的图形:(要求贴图)(1)1,011σμ=时=,-,,在同一坐标系里作图>> syms x;>> fplot('(1/sqrt(2*pi))*exp(-((x)^2)/2)',[-3,3],'r') >> hold on>> fplot('(1/sqrt(2*pi))*exp(-((x-1)^2)/2)',[-3,3],'y') >> hold on>> fplot('(1/sqrt(2*pi))*exp(-((x+1)^2)/2)',[-3,3],'g') >> hold off(2)0,124μσ=时=,,,在同一坐标系里作图。
>> syms x;fplot('(1/sqrt(2*pi))*exp(-((x)^2)/2)',[-3,3],'r') hold onfplot('(1/(sqrt(2*pi)*2))*exp(-((x)^2)/(2*2^2))',[-3,3],'y') hold onfplot('(1/(sqrt(2*pi)*4))*exp(-((x)^2)/(2*4^2))',[-3,3],'g')hold off6、画下列函数的图形:(要求贴图)(1)sin 020cos 024x u tt y u t u tz ⎧⎪=≤≤⎪=⎨≤≤⎪⎪=⎩>>ezmesh('u*sin(t)','u*cos(t)','t/4',[0,20,0,2])(2) sin()03,03z xy x y =≤≤≤≤>> x=0:0.1:3;y=x; [X Y]=meshgrid(x,y); Z=sin(X*Y); >> mesh(X,Y ,Z).9 做一个花瓶,如图示。
(提示:做一个旋转体表面,调入一幅图像对该表面进行彩绘,即用图像的色图索引作为表面体的色图索引) >> t=(0:20)/20; >> r=sin(2*pi*t)+2; >> [x,y,z]=cylinder(r,40); % 产生旋转体表面的三维数据(3)sin (3cos )02cos (3cos )02sin x t u t y t u u z u ππ=+⎧≤≤⎪=+⎨≤≤⎪=⎩ezmesh('sin(t)*(3+cos(u))','cos(t)*(3+cos(u))','sin(u)',[0,2*pi,0,2*pi])7、 已知422134305,203153211A B -⎛⎫⎛⎫ ⎪ ⎪=-=-- ⎪ ⎪ ⎪ ⎪-⎝⎭⎝⎭,在MA TLAB 命令窗口中建立A 、B 矩阵并对其进行以下操作:(1) 计算矩阵A 的行列式的值det()A >> A=[4,-2,2;-3,0,5;1,5,3]; >> det(A)ans =-158(2) 分别计算下列各式:1122,*,.*,,,,T A B A B A B AB A B A A --->> A=[4,-2,2;-3,0,5;1,5,3];B=[1,3,4;-2,0,-3;2,-1,1]; >> 2*A-Bans =7 -7 0-4 0 130 11 5>> A*Bans =12 10 247 -14 -7-3 0 -8>> A.*Bans =4 -6 86 0 -152 -5 3>> A*inv(B)ans =-0.0000 -0.0000 2.0000-2.7143 -8.0000 -8.14292.42863.0000 2.2857>> inv(A)*Bans =0.4873 0.4114 1.00000.3671 -0.4304 0.0000-0.1076 0.2468 0.0000>> A*Aans =24 2 4-7 31 9-8 13 36>> A'ans =4 -3 1-2 0 52 5 3>>8、在MA TLAB中分别利用矩阵的初等变换及函数rank、函数inv求下列矩阵的秩:(1)16323540,11124A-⎛⎫⎪=-⎪⎪--⎝⎭求rank(A)=?>> A=[1,-6,3,2;3,-5,4,0;-1,-11,2,4]; >> rank(A)ans =3(2)35011200,10201202B⎛⎫⎪⎪=⎪⎪⎝⎭求1B-。
>> B=[3,5,0,1;1,2,0,0;1,0,2,0;1,2,0,2]>> inv(B)ans =2.0000 -4.0000 -0.0000 -1.0000-1.0000 2.5000 0.0000 0.5000-1.0000 2.0000 0.5000 0.50000 -0.5000 0 0.50009、在MA TLAB中判断下列向量组是否线性相关,并找出向量组1(1132),Tα=234(1113),(5289),(1317)T T T ααα=--=-=-中的一个最大线性无关组。
>> a1=[1 1 3 2]' a2=[-1 1 -1 3]' a3=[5 -2 8 9]' a4=[-1 3 1 7]'A= [a1, a2 ,a3 ,a4] ;[R jb]=rref(A) a1 =1 1 32 a2 =-1 1 -1 3 a3 =5 -2 8 9 a4 =-1 3 1 7 R =1.0000 0 0 1.09090 1.0000 0 1.78790 0 1.0000 -0.06060 0 0 0jb =1 2 3>> A(:,jb)ans =1 -1 51 1 -23 -1 82 3 910、在MA TLAB中判断下列方程组解的情况,若有多个解,写出通解。
