第三届丘成桐大学生数学竞赛试题-纯数学
大学生数学知识竞赛试题及答案【最新】

趣味数学知识竞赛复习题一、填空题1、(苏步青)是国际公认的几何学权威,我国微分几何派的创始人。
2、(华罗庚)是一个传奇式的人物,是一个自学成才的数学家。
3、编有《三角学》,被称为“李蕃三角”且自称为“三书子”的是(李锐夫)。
4、世界上攻克“哥德巴赫猜想”的第一个人是(陈景润)。
5、(姜立夫)是现代数学在中国最早而又最富成效的播种人”,这是《中国大百科全书》和《中国现代数学家传》对他的共同评价。
6. 设有n个实数,满足|xi|<1(I=1,2,3,…,n), |x1|+|x2|+…+|xn|=19+|x1+x2+…+xn| ,则n的最小值207. 三角形的一个顶点引出的角平分线,高线及中线恰将这个顶点的角四等分,则这个顶角的度数为___90° ___8. 某旅馆有2003个空房间,房间钥匙互不相同,来了2010们旅客,要分发钥匙,使得其中任何2003个人都能住进这2003个房间,而且每人一间(假定每间分出的钥匙数及每人分到的钥匙数都不限),最少得发出_16024______把钥匙.9. 在凸1900边形内取103个点,以这2003个点为顶点,可将原凸1900边形分割成小三角形的个数为______2104 _____.10. 若实数x满足x4+36<13x2,则f(x)=x3-3x的最大值为______18_____11 ."我买鸡蛋时,付给杂货店老板12美分,"一位厨师说道,"但是由于嫌它们太小,我又叫他无偿添加了2只鸡蛋给我。
这样一来,每打(12只)鸡蛋的价钱就比当初的要价降低了1美分。
" 厨师买了_18只鸡蛋?12.已知f(x)∈[0,1],则y=f(x)+1的取值范围为 ___[7/9,7/8]____13. 已知函数f(x)与g(x)的定义域均为非负实数集,对任意的x≥0,规定f(x)*g(x)=min{f(x),g(x)}.若f(x)=3-x,g(x)=,则f(x)*g(x)的最大值为____(2√3-1) _____ 14.已知a,b,cd∈N,且满足342(abcd+ab+ad+cd+1)=379(bcd+b+d),设M=a×103+b×102+c×10+d,则M的值为______ 1949 ___.15. 用E(n)表示可使5k是乘积112233…nn的约数为最大的整数k,则E(150)=__ 2975_________16. 从1到100的自然数中,每次取出不同的两个数,使它们的和大于100,则可有_2500________种不同的取法.17. 从正整数序列1,2,3,4,…中依次划去3的倍数和4的倍数,但是其中是5的倍数均保留,划完后剩下的数依次构成一个新的序列:A1=1,A2=2,A3=5,A4=7,…,则A2003的值为____3338 _____.18. .连接凸五边形的每两个顶点总共可得到十条线段(包括边在内),现将其中的几条线段着上着颜色,为了使得该五边形中任意三个顶点所构成的三角形都至少有一条边是有颜色的则n的最小值是_419. 已知x0=2003,xn=xn-1+ (n>1,n∈N),则x2003的整数部分为_______2003___21. 已知ak≥0,k=1,2,…,2003,且a1+a2+…+a2003=1,则S=max{a1+a2+a3,a2+a3+a4,…, a2001+a2002+a2003}的最小值为________3/2007 _.22. 对于每一对实数x,y,函数f满足f(x)+f(y)=f(x+y)-xy-1,若f(1)=1,那么使f(n)=n(n≠1)的整数n共有_1个.23.在棱长为a的正方体内容纳9个等球,八个角各放一个,则这些等球最大半径是____. (√3-3/2)a ___24.已知a,b,c都不为0,并且有sinx=asin(y-z),siny=bsin(z-x),sinz=csin(x-y).则有ab+bc+ca=__-1 _____.二、选择题1、被誉为中国现代数学祖师的是(1、C )。
大学生数学知识竞赛试题及答案

趣味数学知识竞赛复习题一、填空题1、(苏步青)是国际公认的几何学权威,我国微分几何派的创始人。
2、(华罗庚)是一个传奇式的人物,是一个自学成才的数学家。
3、编有《三角学》,被称为“李蕃三角"且自称为“三书子"的是(李锐夫)。
4、世界上攻克“哥德巴赫猜想”的第一个人是(陈景润).5、( 姜立夫)是现代数学在中国最早而又最富成效的播种人”,这是《中国大百科全书》和《中国现代数学家传》对他的共同评价。
6。
设有n个实数,满足|xi|〈1(I=1,2,3,…,n), |x1|+|x2|+…+|xn|=19+|x1+x2+…+xn|,则n的最小值207。
三角形的一个顶点引出的角平分线,高线及中线恰将这个顶点的角四等分,则这个顶角的度数为___90°___8. 某旅馆有2003个空房间,房间钥匙互不相同,来了2010们旅客,要分发钥匙,使得其中任何2003个人都能住进这2003个房间,而且每人一间(假定每间分出的钥匙数及每人分到的钥匙数都不限),最少得发出_16024______把钥匙.9。
在凸1900边形内取103个点,以这2003个点为顶点,可将原凸1900边形分割成小三角形的个数为______2104 _____。
10。
若实数x满足x4+36<13x2,则f(x)=x3-3x的最大值为______18_____11 .”我买鸡蛋时,付给杂货店老板12美分,"一位厨师说道,”但是由于嫌它们太小,我又叫他无偿添加了2只鸡蛋给我.这样一来,每打(12只)鸡蛋的价钱就比当初的要价降低了1美分。
”厨师买了_18只鸡蛋?12.已知f(x)∈[0,1],则y=f(x)+1的取值范围为___[7/9,7/8]____13。
已知函数f(x)与g(x)的定义域均为非负实数集,对任意的x≥0,规定f(x)*g(x)=min{f(x),g(x)}.若f(x)=3-x,g(x)=,则f(x)*g(x)的最大值为____(2√3-1)_____14.已知a,b,cd∈N,且满足342(abcd+ab+ad+cd+1)=379(bcd+b+d),设M=a×103+b ×102+c×10+d,则M的值为______ 1949 ___.15. 用E(n)表示可使5k是乘积112233…nn的约数为最大的整数k,则E(150)= __2975_________16. 从1到100的自然数中,每次取出不同的两个数,使它们的和大于100,则可有_2500________种不同的取法.17。
丘成桐数学竞赛试卷小学

一、选择题(每题5分,共20分)1. 小明有5个苹果,小红有8个苹果,他们两个一共有多少个苹果?A. 13个B. 15个C. 20个D. 18个2. 小猫吃鱼的速度是小狗的2倍,如果小狗吃鱼需要10分钟,那么小猫吃鱼需要多少分钟?A. 5分钟B. 10分钟C. 15分钟D. 20分钟3. 一个长方形的长是8厘米,宽是4厘米,它的周长是多少厘米?A. 16厘米B. 24厘米C. 32厘米D. 40厘米4. 小明今年8岁,他的爸爸比他大20岁,那么他爸爸今年多少岁?A. 28岁B. 29岁C. 30岁D. 31岁5. 一个正方形的边长是6厘米,它的面积是多少平方厘米?A. 36平方厘米B. 48平方厘米C. 54平方厘米D. 60平方厘米二、填空题(每题5分,共20分)6. 如果一个数的3倍加上4等于14,那么这个数是______。
7. 一个三角形的三边长分别是3厘米、4厘米、5厘米,这是一个______三角形。
8. 一个圆的半径是7厘米,它的直径是______厘米。
9. 小华有15个橘子,他每天吃3个,他需要______天才能吃完。
10. 一个长方形的长是10厘米,宽是5厘米,它的面积是______平方厘米。
三、解答题(每题10分,共30分)11. 小明有12个气球,他给了小红6个,然后又给了小刚4个,现在小明还剩多少个气球?12. 小明家的花园是一个长方形,长是20米,宽是10米,请问花园的面积是多少平方米?13. 小红有一些硬币,其中5分硬币有8个,1角硬币有5个,2角硬币有3个,小红一共有多少角硬币?四、应用题(每题10分,共20分)14. 小丽有一些铅笔,她每天用掉2支,用了5天后,她剩下8支铅笔,请问小丽原来有多少支铅笔?15. 小明和小华一起收集邮票,小明有40张,小华有60张,他们两个一共有多少张邮票?---注意:本试卷为模拟试题,旨在帮助小学生练习数学知识。
答案仅供参考,实际竞赛题目可能会有所不同。
第三届全国大学生数学竞赛决赛试题非数学类部分

个人采集整理 仅供参照学习第三届全国大学生数学比赛决赛试卷(非数学类, 2012)本试卷共 2 页,共 6 题。
全卷满分 100 分。
考试用时 150 分钟。
一、(本大题共 5 小题,每题 6 分,共 30 分)计算以下各题(要求写出重要步骤) .(1) limsin 2 x x 2 cos 2 x22x 0x sin x(2) limx31 x tan 111 x6e xx2 x(3) 设函数 f (x , y) 有二阶连续偏导数 , 知足 f x 2 f yy 2 f x f y f xy f y 2 f yy 0 且f y0 , y y( x , z) 是由方程 z2yf (x , y) 所确立的函数 . 求x 2(4)求不定积分 I(1x x11)exdxx(5) 求曲面 x 2 y 2az 和 z 2ax 2 y 2 (a 0) 所围立体的表面积二、(此题 13 分)议论xdx 的敛散性,此中是一个实常数 .cos 2 xx sin 2 x得分三、(此题 13 分)设 f (x) 在 ( ,) 上无量次可微,而且知足 :存在 M 0 ,使得 f( k )(x)M , x (,),( k 1,2 ) , 且 f (1n ) 0,( n1,2 ) 求 证 : 在2( ,上), f ( x) 0四、(此题共 16分,第 1小题 6分,第 2小题 10分)设 D 为椭圆形x 2y 2 1(a b 0) ,面密度为 ρ的均质薄板; l 为经过椭圆焦点a 2b 2( c,0) (此中 c 2a 2b 2 )垂直于薄板的旋转轴 .1. 求薄板 D 绕l 旋转的转动惯量 J ;2. 关于固定的转动惯量,议论椭圆薄板的面积能否有最大值和最小值.五、(此题 12 分)设连续可微函数 z f (x, y) 由方程 F ( xz y, x yz)0 (此中F (u, v) 0 有 连 续 的 偏 导 数 )唯 一 确 定 , L 为 正 向单 位 圆 周 . 试 求 :个人采集整理仅供参照学习I( xz 22 yz)dy (2 xz yz 2 )dxL解:由格林公式I( xz22 yz)dy (2 xz yz 2) dx( Q P )dLDx y( z22xzz2 y z ) (2 xzz 22 yz z)d 2 z 22( xz y)z2( x yz) zdD x x y y Dxy又:连续可微函数 z f (x, y) 由方程 F ( xz y, x yz) 0两边同时对 x 求偏导数: F 1 (zxz F 2 (1z) 0z zF 1 F 2 )yx yF 2 xF 1xx两边同时对 y 求偏导数: F 1 ( x z 1)F 2 ( z y z ) 0zF 1 zF 2yy xxF 1 yF 2代入上式:六、(此题共 16 分,第 1 小题 6 分,第 2 小题 10 分)(1)求解微分方程y xy xe x 2y(0)11 nf (x)dx(2)如 y f ( x) 为上述方程的解,证明 lim22n1 n x2。
清华丘成桐大学生数学竞赛2021年笔试真题computational_and_applied_21s
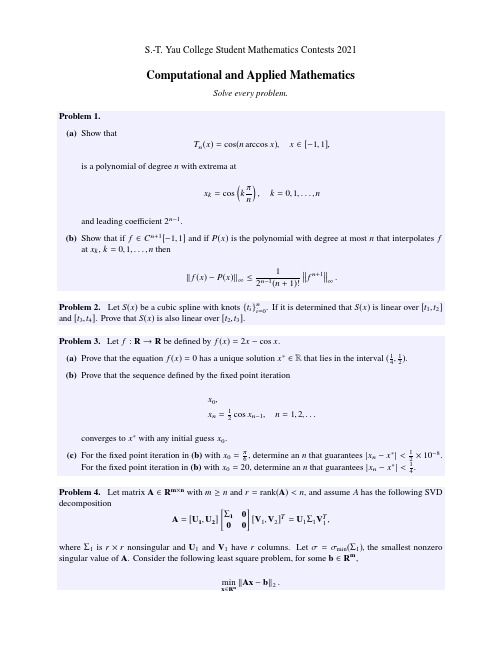
min
x ∈Rn
Ax − b 2 .
(a) Show that all solutions x can be written as
x = V1Σ−1 1UT1 b + V2z2,
with z2 an arbitrary vector. (b) Show that the solution x has minimal norm x 2 precisely when z2 = 0, and in which case,
=
π 6
,
determine
an
n
that
guarantees
|
xn
−
x∗|
<
1 2
× 10−8.
For
the
fixed
point
iteration
in
(b)
with
x0
=
20,
determine
an
n
that
guarantees
| xn
−
x∗|
<
1 4
.
Problem 4. Let matrix A ∈ Rm×n with m ≥ n and r = rank(A) < n, and assume A has the following SVD
(e) Prove an a priori error estimate for this method in the L2 norm:
∫1
1 2
e L2 =: e =
e2dx .
0
(b) Prove that the sequence defined by the fixed point iteration
丘成桐小学数学竞赛试卷

一、选择题(每题5分,共20分)1. 下列哪个数是偶数?A. 7B. 10C. 15D. 222. 小明有5个苹果,小华有3个苹果,他们一共有多少个苹果?A. 8B. 10C. 12D. 153. 一个长方形的长是6厘米,宽是4厘米,它的周长是多少厘米?A. 10B. 16C. 20D. 244. 一个正方形的边长是8厘米,它的面积是多少平方厘米?A. 16B. 32C. 64D. 1285. 小刚有20元,他买了一本书用了12元,他还剩下多少钱?A. 8C. 12D. 20二、填空题(每题5分,共20分)6. 2乘以5等于______。
7. 3加4等于______。
8. 8减去3等于______。
9. 一个正方形的面积是36平方厘米,它的边长是______厘米。
10. 一个长方形的周长是26厘米,如果它的长是8厘米,那么它的宽是______厘米。
三、解答题(每题10分,共30分)11. 小红有苹果、香蕉和橙子一共30个,苹果比香蕉多10个,香蕉比橙子多5个。
请问小红分别有多少个苹果、香蕉和橙子?12. 一个长方形的长是15厘米,宽是10厘米,如果将这个长方形剪成两个正方形,那么每个正方形的边长是多少厘米?13. 小明骑自行车去图书馆,往返共用了40分钟。
去图书馆用了20分钟,那么他骑自行车去图书馆的速度是每分钟多少米?四、应用题(每题15分,共30分)14. 小明去书店买书,他带的钱是100元。
一本故事书20元,一本科学书30元,一本漫画书25元。
小明想买一本故事书和一本漫画书,他带的钱够不够?15. 小花家的花园长10米,宽8米。
她在花园里种了20棵苹果树,每棵树需要2平方米的空间。
请问小花还剩下多少平方米的空间种其他植物?答案:一、选择题1. B3. C4. C5. B二、填空题6. 107. 78. 59. 610. 6三、解答题11. 苹果15个,香蕉5个,橙子10个。
12. 每个正方形的边长是5厘米。
丘成桐大学生数学竞赛试卷

S.-T.Yau College Student Mathematics Contests 2010Analysis and Differential EquationsTeam(Please select 5problems to solve)1.a)Let f (z )be holomorphic in D :|z |<1and |f (z )|≤1(z ∈D ).Prove that|f (0)|−|z |1+|f (0)||z |≤|f (z )|≤|f (0)|+|z |1−|f (0)||z |.(z ∈D )b)For any finite complex value a ,prove that 12π 2π0log |a −e iθ|dθ=max {log |a |,0}.2.Let f ∈C 1(R ),f (x +1)=f (x ),for all x ,then we have ||f ||∞≤ 10|f (t )|dt + 10|f (t )|dt.3.Consider the equation¨x +(1+f (t ))x =0.We assume that ∞|f (t )|dt <∞.Study the Lyapunov stability of the solution (x,˙x )=(0,0).4.Suppose f :[a,b ]→R be a L 1-integrable function.Extend f to be 0outside the interval [a,b ].Letφ(x )=12h x +h x −hf Show thatb a |φ|≤ b a |f |.5.Suppose f ∈L 1[0,2π],ˆf (n )=12π 2π0f (x )e −inx dx ,prove that 1)∞ |n |=0|ˆf(n )|2<∞implies f ∈L 2[0,2π],2)n |n ˆf (n )|<∞implies that f =f 0,a.e.,f 0∈C 1[0,2π],where C 1[0,2π]is the space of functions f over [0,1]such that both f and its derivative f are continuous functions.126.SupposeΩ⊂R3to be a simply connected domain andΩ1⊂Ωwith boundaryΓ.Let u be a harmonic function inΩand M0=(x0,y0,z0)∈Ω1.Calculate the integral:II=−Γu∂∂n(1r)−1r∂u∂ndS,where 1r=1(x−x0)2+(y−x0)2+(z−x0)2and∂∂ndenotes theout normal derivative with respect to boundaryΓof the domainΩ1.(Hint:use the formula∂v∂n dS=∂v∂xdy∧dz+∂v∂ydz∧dx+∂v∂zdx∧dy.)S.-T.Yau College Student Mathematics Contests 2010Applied Math.,Computational Math.,Probability and StatisticsTeam(Please select 5problems to solve)1.Let X 1,···,X n be independent and identically distributed random variables with continuous distribution functions F (x 1),···,F (x n ),re-spectively.Let Y 1<···<Y n be the order statistics of X 1,···,X n .Prove that Z j =F (Y j )has the beta (j,n −j +1)distribution (j =1,···,n ).2.Let X 1,···,X n be i.i.d.random variable with a continuous density f at point 0.LetY n,i =34b n (1−X 2i /b 2n )I (|X i |≤b n ).Show that n i =1(Y n,i −EY n,i )(b n n i =1Y n,i )1/2L −→N (0,3/5),provided b n →0and nb n →∞.3.Let X 1,···,X n be independently and indentically distributed ran-dom variables with X i ∼N (θ,1).Suppose that it is known that |θ|≤τ,where τis given.Showmin a 1,···,a n +1sup |θ|≤τE (n i =1a i X i +a n +1−θ)2=τ2n −1τ2+n −1.Hint:Carefully use the sufficiency principle.4.The rules for “1and 1”foul shooting in basketball are as follows.The shooter gets to try to make a basket from the foul line.If he succeeds,he gets another try.More precisely,he make 0baskets by missing the first time,1basket by making the first shot and xsmissing the second one,or 2baskets by making both shots.Let n be a fixed integer,and suppose a player gets n tries at “1and 1”shooting.Let N 0,N 1,and N 2be the random variables recording the number of times he makes 0,1,or 2baskets,respectively.Note that N 0+N 1+N 2=n .Suppose that shots are independent Bernoulli trails with probability p for making a basket.(a)Write down the likelihood for (N 0,N 1,N 2).12(b)Show that the maximum likelihood estimator of p is ˆp =N 1+2N 2N 0+2N 1+2N 2.(c)Is ˆp an unbiased estimator for p ?Prove or disprove.(Hint:E ˆp is a polynomial in p ,whose order is higher than 1for p ∈(0,1).)(d)Find the asymptotic distribution of ˆp as n tends to ∞.5.When considering finite difference schemes approximating partial differential equations (PDEs),for example,the scheme(1)u n +1j =u n j −λ(u n j −u n j −1)where λ=∆t ∆x ,approximating the PDE (2)u t +u x =0,we are often interested in stability,namely(3)||u n ||≤C ||u 0||,n ∆t ≤T for a constant C =C (T )independent of the time step ∆t and the spa-tial mesh size ∆x .Here ||·||is a given norm,for example the L 2norm orthe L ∞norm,of the numerical solution vector u n =(u n 1,u n 2,···,u n N ).The mesh points are x j =j ∆x ,t n =n ∆t ,and the numerical solutionu n j approximates the exact solution u (x j ,t n )of the PDE (2)with aperiodic boundary condition.(i)Prove that the scheme (1)is stable in the sense of (3)for boththe L 2norm and the L ∞norm under the time step restriction λ≤1.(ii)Since the numerical solution u n is in a finite dimensional space,Student A argues that the stability (3),once proved for a spe-cific norm ||·||a ,would also automatically hold for any other norm ||·||b .His argument is based on the equivalency of all norms in a finite dimensional space,namely for any two norms ||·||a and ||·||b on a finite dimensional space W ,there exists a constant δ>0such thatδ||u ||b ≤||u ||a ≤1δ||u ||b .Do you agree with his argument?If yes,please give a detailed proof of the following theorem:If a scheme is stable,namely (3)holds for one particular norm (e.g.the L 2norm),then it is also stable for any other norm.If not,please explain the mistake made by Student A.6.We have the following 3PDEs(4)u t +Au x =0,(5)u t +Bu x =0,3 (6)u t+Cu x=0,C=A+B.Here u is a vector of size m and A and B are m×m real matrices. We assume m≥2and both A and B are diagonalizable with only real eigenvalues.We also assume periodic initial condition for these PDEs.(i)Prove that(4)and(5)are both well-posed in the L2-norm.Recall that a PDE is well-posed if its solution satisfies||u(·,t)||≤C(T)||u(·,0)||,0≤t≤Tfor a constant C(T)which depends only on T.(ii)Is(6)guaranteed to be well-posed as well?If yes,give a proof;if not,give a counter example.(iii)Suppose we have afinite difference schemeu n+1=A h u nfor approximating(4)and another schemeu n+1=B h u nfor approximating(5).Suppose both schemes are stable in theL2-norm,namely(3)holds for both schemes.If we now formthe splitting schemeu n+1=B h A h u nwhich is a consistent scheme for solving(6),is this scheme guar-anteed to be L2stable as well?If yes,give a proof;if not,givea counter example.S.-T.Yau College Student Mathematics Contests2010Geometry and TopologyTeam(Please select5problems to solve)1.Let S n⊂R n+1be the unit sphere,and R n⊂R n+1the equator n-plane through the center of S n.Let N be the north pole of S n.Define a mappingπ:S n\{N}→R n called the stereographic projection that takes A∈S n\{N}into the intersection A ∈R n of the equator n-plane R n with the line which passes through A and N.Prove that the stereographic projection is a conformal change,and derive the standard metric of S n by the stereographic projection.2.Let M be a(connected)Riemannian manifold of dimension2.Let f be a smooth non-constant function on M such that f is bounded from above and∆f≥0everywhere on M.Show that there does not exist any point p∈M such that f(p)=sup{f(x):x∈M}.3.Let M be a compact smooth manifold of dimension d.Prove that there exists some n∈Z+such that M can be regularly embedded in the Euclidean space R n.4.Show that any C∞function f on a compact smooth manifold M (without boundary)must have at least two critical points.When M is the2-torus,show that f must have more than two critical points.5.Construct a space X with H0(X)=Z,H1(X)=Z2×Z3,H2(X)= Z,and all other homology groups of X vanishing.6.(a).Define the degree deg f of a C∞map f:S2−→S2and prove that deg f as you present it is well-defined and independent of any choices you need to make in your definition.(b).Prove in detail that for each integer k(possibly negative),there is a C∞map f:S2−→S2of degree k.1S.-T.Yau College Student Mathematics Contests 2010Algebra,Number Theory andCombinatoricsTeam(Please select 5problems to solve)1.For a real number r ,let [r ]denote the maximal integer less or equal than r .Let a and b be two positive irrational numbers such that 1a +1b = 1.Show that the two sequences of integers [ax ],[bx ]for x =1,2,3,···contain all natural numbers without repetition.2.Let n ≥2be an integer and consider the Fermat equationX n +Y n =Z n ,X,Y,Z ∈C [t ].Find all nontrivial solution (X,Y,Z )of the above equation in the sense that X,Y,Z have no common zero and are not all constant.3.Let p ≥7be an odd prime number.(a)Evaluate the rational number cos(π/7)·cos(2π/7)·cos(3π/7).(b)Show that (p −1)/2n =1cos(nπ/p )is a rational number and deter-mine its value.4.For a positive integer a ,consider the polynomialf a =x 6+3ax 4+3x 3+3ax 2+1.Show that it is irreducible.Let F be the splitting field of f a .Show that its Galois group is solvable.5.Prove that a group of order 150is not simple.6.Let V ∼=C 2be the standard representation of SL 2(C ).(a)Show that the n -th symmetric power V n =Sym n V is irre-ducible.(b)Which V n appear in the decomposition of the tensor productV 2⊗V 3into irreducible representations?1。
丘成桐大学生数学竞赛2021年笔试真题probability_and_statistics_21s
S.-T.Yau College Student Mathematics Contests2021Probability and StatisticsSolve every problem.Part I:ProbabilityProblem1.Suppose that a sequence{X n}of real-valued random variables converges to X in distribution and there are positive constants r and C such that E|X n|r≤C for all n.Show thatlimn→∞E|X n|s=E|X|sfor all0<s<r.Problem2.Let p(x,y)be the(one-step)transition function of a Markov chain on a discrete state space S and p n(x,y)be the n-step transition function.Show that for any positive integers L and N and any two states x and y we haveN+Ln=L p n(x,y)≤Nn=0p n(y,y).Part II:StatisticsProblem5.You have been asked to help design a randomized trial of a new drug,call it drug A,to be used in place of the current drug,call it drug B,for a particular medical condition.The budget isfixed to have 1000patients treated with A and1000treated with drug B.The issue is how to do the allocation of patients, because we have many pre-randomization measurements on each patient,roughly200,such as blood pressurerecordings,age,sex,and a large collection of genetics measurements.Obviously it is desirable to have the A group similar to the B group with respect to all pre-treatment covariates and non-linear functions of them that are expected to influence the effectiveness of the drugs with respect to the outcome variables.Complete(or simple)randomization does this in expectation,but with many covariates,some covariates will not be balanced between the A and B groups in any one single randomized allocation.Standard blocking used in traditional experimental design can force balance on a few covariates,but the designer of drug A wants to have an experimental design that creates balance on many covariates,and feels that you,as a modern applied mathematician/statistician,should be able to do this.Describe a class of methods that achieves this goal where each patient has a positive probability of receiving drug A and a positive probability of receiving drug B.Provide enough detail that you are describing an explicit algorithm.Problem6.You are given the results of a randomized experiment of two drugs,A and B.The experiment was not conducted in the usual way,however,but rather by allocating patients by a machine-learning algorithm under which each patient has a positive probability of receiving A and of receiving B;moreover the algorithm is completely specified and is built to create better than random balance on the covariates.(a)Can unbiased estimates of the causal effect of drug A versus B be found,and if so,show why.(b)Can exact small sample,non-parametric inferences for the causal effect in part(a)be derived,basedsolely on the randomization distribution of some statistic?For example,can wefind exact significance levels under a sharp null hypothesis?If so,outline how to accomplish this goal.。
全国大学生数学竞赛赛试题(1-9届)

一、填空题(每小题5分,共20分)1.计算=--++⎰⎰y x yx x yy x Dd d 1)1ln()(__ ,其中区域D 由直线1=+y x 与两坐标轴所围成三角形区域. 2.设)(x f 是连续函数,且满足⎰--=222d )(3)(x x f x x f , 则=)(x f ____________.3.曲面2222-+=y x z 平行平面022=-+z y x 的切平面方程是__________. 4.设函数)(x y y =由方程29ln )(yy f e xe=确定,其中f 具有二阶导数,且1≠'f ,则=22d d xy_____.二、(5分)求极限xenx x x x ne e e )(lim 20+++→Λ,其中n 是给定的正整数.三、(15分)设函数)(x f 连续,⎰=10d )()(t xt f x g ,且A xx f x =→)(lim,A 为常数,求)(x g '并讨论)(x g '在0=x 处的连续性.四、(15分)已知平面区域}0,0|),{(ππ≤≤≤≤=y x y x D ,L 为D 的正向边界,试证:(1)⎰⎰-=---Lx y L x y x ye y xe x ye y xe d d d d sin sin sin sin ; (2)2sin sin 25d d π⎰≥--L y y x ye y xe .五、(10分)已知x x e xe y 21+=,x x e xe y -+=2,x x x e e xe y --+=23是某二阶常系数线性非齐次微分方程的三个解,试求此微分方程.六、(10分)设抛物线c bx ax y ln 22++=过原点.当10≤≤x 时,0≥y ,又已知该抛物线与x 轴及直线1=x 所围图形的面积为31.试确定c b a ,,,使此图形绕x 轴旋转一周而成的旋转体的体积最小.七、(15分)已知)(x u n 满足),2,1()()(1Λ=+='-n e x x u x u xn n n, 且n eu n =)1(, 求函数项级数∑∞=1)(n n x u 之和. 八、(10分)求-→1x 时, 与∑∞=02n n x 等价的无穷大量.一、(25分,每小题5分)(1)设22(1)(1)(1),n n x a a a =+++L 其中||1,a <求lim .n n x →∞(2)求21lim 1x x x e x -→∞⎛⎫+ ⎪⎝⎭。
第三届全国大学生数学竞赛决赛试题(非数学类)+答案

第三届全国大学生数学竞赛决赛试题(非数学类)+答案第三届全国大学生数学竞赛决赛试卷(非数学类,2012)本试卷共2页,共6题。
全卷满分100分。
考试用时150分钟。
一、(本大题共5小题,每小题6分,共30分)计算下列各题(要求写出重要步骤).(1) xx xx x x 222220sin cos sin lim -→解:x x x x x x 222220sin cos sin lim -→4222220cos sin lim x xx x x x x -+-=→2040)c o s 1)(cos 1(lim ))(sin (sin lim x x x x x x x x x x +-++-=→→221261?+?-=32=(2) [()]61311tan 21lim x e xx x x x +--++∞→解: [()]61311tan 21lim x e xx x xx +--++∞→ (令x t 1=)362201)t a n 21(l i m t t e t t t t t +--+=+→3620111)21(lim t t e t t t +-+-+=+→ 3201)21(l i m t e t tt -+=+→2206)22(lim te t t t t ++=+→+∞=(3) 设函数),(y x f 有二阶连续偏导数, 满足0222=+-yy y xy y x yy x f f f f f f f 且0≠y f ,),(z x y y =是由方程),(y x f z =所确定的函数. 求22xy解:依题意有,y 是函数,x 、z 是自变量。
将方程),(y x f z =两边同时对x 求导, x y ffyx+=0,则 yx f f x y-=??,于是 ()yx f f x x y -=??222)()(yyy yx x yxxx y f x yf f f x y f f f ??+-??+-=2)()(yyx yy yx x yx yxxx y f f f f f f f f f f f ----=3222yyyy xy y x yy x f f f f f f f f +--=0=(4) 求不定积分()dx e xx I x x 111+-+=?解:()dx e x x dx eI xx xx 12111++-+=?xx x x xdedx e 11+++=?()xx xe d 1+?=C xexx +=+1(5) 求曲面az y x =+22和222y x a z +-=)0(>a 所围立体的表面积解:联立az y x =+22,222y x a z +-=,解得两曲面的交线所在的平面为a z =,它将表面分为1S 与2S 两部分,它们在xoy 平面上的投影为222:a y x D ≤+,在1S 上 dxdy a y a x dS 2222441++=dxdy a y x a 2222)(4++=在2S 上 dxdy yx y y x x dS 2222221++++=dxdy 2= 则 d x d y ay x a S D )2)(4(2222+++=??22202024a r d r a r a d a πθπ+=?? )26155(2+-=a π 二、(本题13分)讨论dx xx x x220sin cos α+?∞+的敛散性,其中α是一个实常数. 解:记 xx x xx f 22sin cos )(α+=① 若0≤α,)1(2)(>?≥x xx f ;则dx x x x x 220sin cos α+?∞+发散② 若20≤<α,则11≤-α,而)1(2)(1≥?≥-x x x f α;所以dx xx x x220sin cos α+?∞+发散。
清华丘成桐大学生数学竞赛2021年笔试真题algebra_and_numbertheory_21s

Problem 6. Let p be a prime number and Qp be the field of p-adic numbers. Fix an algebraic closure Qp of Qp. Let g : Z≥0 → N be a strictly increasing function. For each i ∈ Z≥0, pick a primitive (pg(i) − 1)-th
S.-T. Yau College Student Mathematics Contests 2021
Algebra and Number Theory
Solve every problem.
Problem 1. For any prime p and a nonzero element a ∈ Fp, prove that the polynomial A(x) = xp − x − a is irreducible and separable over Fp.
be an increasing sequence of nonnegative integers. Let αi
i j=0
ζj
pNj
.
Show that for each i
≥
0,
Ki = Qp(αi) and that (αi) is a Cauchy sequence in Qp.
(c) Let η ∈ Qp be of degree g over Qp, prove that there exists M ∈ N such that ζi does not satisfy any
Problem 2. Determine the automorphism group of the splitting field of f (x) = x3 − 3x + 1 over Q.
丘成桐班初试题

18. 2023 GDFZ丘成桐班初试时间:80分钟满分:120分一、填空题(每小题6分,共60分)1.(量率对应)甲、乙、丙、丁四人去买电视机,甲带的钱是另外三人所带钱总数的一半,乙带的钱是另外三人所带的钱总数的13,丙带的钱是另外三人所带总钱数的14,丁带910元,四人所带的总钱数是___________元。
2.(方程的应用)两人从甲地到乙地同时出发,一人用匀速3小时走完全程,另一人用匀速4小时走完全程,经过___________小时,其中一人所剩路程的长是另一人所剩路程的长的2倍。
3.(割补法求面积)如图,直角梯形ABCD中四边形AEGF、MBKN都是正方形,且AE=MB,EP=KC=9,DF=PM= 4,则△DPC的面积为__________.4.(分数的应用)今有桃95个,分给甲、乙两班学生吃,甲班分到的桃有29坏的,其他是好的,乙班分到的桃有316是坏的,其他是好的,甲、乙两班分到的好桃共有________个。
5.(和差法求面积)如图,ABCD是平行四边形,AD=8cm,AB=10cm,∠DAB=30°,高CH=4m,弧BE,DF分别以AB,CD为半径,弧D M,BN分别以AD,CB为半径,阴影部分的面积为__________.6.(钟表问题)如图,假设某星球的一天只有6小时,每小时36分钟,那么3点18分时,时针和分针所形成的锐角是__________度。
7.(接送问题)甲、乙、丙三人同时从A地出发到距A地100千米的B地,甲与丙以25千米/时的速度驾车行进,而乙却以5千米/时的速度步行,过了一段时间后,丙下车改以5千米/时的速度步行,而甲驾车以原速折回,将乙载上后前往B地,这样甲、乙、丙三人同时到达B地,此旅程共用时为__________小时。
8.(最值问题)已知A、B、C、D、E、F、G、H、I、J、K代表十个互不相同的大于0的自然数,要使下列等式成立。
A最小是__________。
数学竞赛-完全平方数

(3m+1)=9+6m+1=3k+1
(3m+2)=9+12m+4=3k+1 同理可以得到:
性质 7:不能被 5 整除的数的平方为 5k±1 型,能被 5 整除的数的平方为 5k 型。 性质 8:平方数的形式具有下列形式之一:16m,16m+1,16m+4,16m+9。 除了上面关于个位数,十位数和余数的性质之外,还可研究完全平方数各位数字之 和。例如,256 它的各位数字相加为 2+5+6=13,13 叫做 256 的各位数字和。如果再 把 13 的各位数字相加:1+3=4,4 也可以叫做 256 的各位数字的和。下面我们提到 的一个数的各位数字之和是指把它的各位数字相加,如果得到的数字之和不是一位 数,就把所得的数字再相加,直到成为一位数为止。我们可以得到下面的命题: 一个数的数字和等于这个数被 9 除的余数。 下面以四位数为例来说明这个命题。 设四位数为,则
中符合题意的只有 2401 一个。 [例 11]:求自然数 n,使的值是由数字 0,2,3,4,4,7,8,8,9 组成。 解:显然,。为了便于估计,我们把的变化范围放大到,于是,即。∵,∴。
另一方面,因已知九个数码之和是 3 的倍数,故及 n 都是 3 的倍数。这样,n 只有 24,27,30 三种可能。但 30 结尾有六个 0,故 30 不合要求。经计算得
k=1
k=2
k=3
4
k=5 所以此自然数为 1369, 2601, 3481, 5329, 6561, 9025。
[例 8]:甲、乙两人合养了 n 头羊,而每头羊的卖价又恰为 n 元,全部卖完后, 两人分钱方法如下:先由甲拿十元,再由乙拿十元,如此轮流,拿到最后,剩下不 足十元,轮到乙拿去。为了平均分配,甲应该补给乙多少元(第 2 届“祖冲之杯”初中 数学邀请赛试题)?
丘成桐初中数学竞赛题目

丘成桐初中数学竞赛题目
1.求一个三位数,它的个位数字等于百位数字,它的十位数字是个位数字加上十位数字的和的一半。
2. 某班有40名学生,其中男生人数是女生人数的2倍。
如果班级男生人数增加了10人,女生人数减少了10人,那么男女生人数的比例是多少?
3. 一个数除以5余1,除以6余5,除以7余4,那么这个数是多少?
4. 在平面直角坐标系中,点A(-2,1)和点B(5,-4)的中点是点C。
点D在y轴上,且点D到点C的距离等于点A到点B的距离,求点D 的坐标。
5. 某个数字除以7余3,如果把这个数字的十位数和个位数交换位置,得到的新数字除以7的余数是多少?
6. 在一个矩形中,长比宽多5米,对角线长15米,求这个矩形的面积。
7. 一条直线y=kx+1过点(2,3),如果在这条直线上再找一点,使这两个点到直线x轴的距离之和等于10,求这个点的坐标。
8. 已知正整数a,b,c满足a^2+b^2+c^2=99,且a>b>c,求a,b,c的值。
9. 某个数除以3余2,除以4余3,除以5余4,那么这个数最小是多少?
10. 有两个容器,分别可以装6升和8升水,没有刻度尺,可以
借助这两个容器来称量4升水,问怎么做?。
第三届丘成桐大学生数学竞赛试题-纯数学

(1) Show that either R = Z or have rank 2 over Z.
1
2
INDIVIDUAL TEST
(2) Let n ≥ 3 be a positive integer and (R/nR)× the group of invertible elements in the quotient R/nR. Show that the canonical group homomorphism
1
INDIVIDUAL TEST S.-T YAU COLLEGE MATH CONTESTS 2012
Geometry and Topology
Please solve 5 out of the following 6 problems, or highest scores of 5 problems will be counted.
C is a simple (that is, without self-intersection), smooth, closed curve
in the Euclidean plane, with length L. Show that the area enclosed by
C
is
less
than
U ⊂ M satisfying that [Xi, Xj] = 0 for 1 ≤ i, j ≤ k. Prove that for any point p ∈ U there is a coordinate chart (V, yi) with p ∈ V ⊆ U and
coordinates {y1, · · · , yn} such that Xi =
第三届丘成桐大学生数学竞赛试题-概率统计及应用数学

(c) Find the density of (U1 · · · Un)−X.
INDIVIDUAL TEST S.-T YAU COLLEGE MATH CONTESTS 2012
Applied Math. and Computational Math.
Please solve 4 out of the following 5 problems.
if γ = 0.5.
Assume that there are no tied data values.
(a)
Show
that
W
=
U
+
1 2
n(n
+
1),
which
shows
that
the
two
test
statistics differ only by a constant and yield exactly the same
(a) prove that there is a unique solution P x(x) to (2) (b) denote h = maxk |xk − x|, prove
|ci
−
1 i!
f
(i)(x)|
=
C (f ,
i)hm+1−i,
i = 0, 1, . . . , m,
where f (i)(·) is the i-th derivative of f and C(f, i) denote some constant
1. In the numerical integration formula
1
(1)
f (x)dx ≈ af (−1) + bf (c),
第三届全国大学生数学竞赛(数学类)

第 3 页 ( 共 13页 )
四 、 (本题 10 分) 对于 ∆ABC , 求 3 sin A + 4 sin B + 18 sin C 的最大值. 解 答: 三角形三个角 A, B, C 的取值范围为 (A, B, C ) ∈ D ≡ {(α, β, γ )|α + β + γ = π, α > 0, β > 0, γ > 0} . 我们首先考虑 3 sin A + 4 sin B + 18 sin C 在 D 的闭包 E = {(α, β, γ )|α + β + γ = π, α ≥ 0, β ≥ 0, γ ≥ 0} 上的最大值. 我们有 max (3 sin A + 4 sin B + 18 sin C ) ....................................................... (1 分)
..................................................................... 可以有很多种方法选取只取值 ±1 的数列 {an }n≥1 使得
n ∑ ak √ = α. lim n→+∞ 2 n k=1
(5 分)
此时就成立
n→+∞
n (∑ ) √ 3 lim n + ak − n 2 = α. k=1
0
ak
/
1 ∑ fk (x) dx = 1. n k=1 ak
n
.................................................................... 由此立即可得存在 ξ ∈ [0, 1] 使得
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
Analysis and Differential Equations
Please solve 5 out of the following 6 problems, or highest scores of 5 problems will be counted.
g
=
[1 +
ni=1(dxi)2
c 4
n i=1
(xi)2
]2
where (x1, . . . , xn) is a local coordinate system.
1
INDIVIDUAL TEST
S.-T YAU COLLEGE MATH CONTESTS 2012
5.
For
1
<
p, q
<
∞,
1 p
+
1 q
=
1,
let
g
in
Lq .
Consider the linear
functional F on Lp given by: F (f ) is equal to the integral of f g. Show
that ||F || = ||g||q.
6. Let Rn+ = {x = (x1, x2, ..., xn) ∈ Rn|xn > 0}. Show that the formula
3. Let f (x) be a C2 function on R. Show that sup |f (x)|2 ≤ 4 sup |f (x)| sup |f (x)|.
4. Let f (x) be a real measurable function defined on [a, b]. Let n(y) be the number of solutions of the equation f (x) = y. Prove that n(y) is a measurable function on R.
1. Compute the integral
∞ 0
1
xp + x2
dx,
−1
<
p
<
1.
2. Construct a one to one conformal mapping from the region
U
=
{z
∈
C||z
−
i 2
|
<
1 2
}/{z||z
−
i 4
|
<
1 4
}
onto the unit disk.
∂ ∂yi
on V
for each 1 ≤ i ≤ k.
3. Show that any self homeomorphism of CP2 is orientation preserving.
4. Prove the following version of the isoperimetric inequality: Suppose
or
equal
to
L2 4π
,
and
the
equality
curs
when
and
only
when C is a round circle.
5. Let x : M → R3 be a closed surface in 3-dimensional Euclidean space. Its Gaussian curvature and mean curvature are denoted by K and H respectively. Prove that:
u(x)
=
2xn nαn
∂Rn+
g(y) |x − y|n
dy,
x
∈
Rn+
is a solution of the problem
∆u = 0, in Rn+, u = g on ∂Rn+, where αn is the volume of the unit n dimensional sphere.
U ⊂ M satisfying that [Xi, Xj] = 0 for 1 ≤ i, j ≤ k. Prove that for any point p ∈ U there is a coordinate chart (V, yi) with p ∈ V ⊆ U and
coordinates {y1, · · · , yn} such that Xi =
C is a simple (that is, without self-intersection), smooth, closed curve
in the Euclidean plane, with length L. Show that the area enclosed by
C
is
less
than
element of M .
6. Write the structure equation of an orthonormal frame on a Riemann-
ian manifold. Prove the following Riemannian metric g has constant
sectional curvature c using the structure equation:
HdA + pKdA = 0,
pHdA + dA = 0,
M
M
M
M
where p = x · n is the support function of M , x denotes the position
vector of M , n denotes the unit normal to M , and dA is the area
1. Show that π3(S2) = 0.
2. Let M be a smooth manifold of dimension n, and X1, · · · , Xk be k everywhere linearly independent smooth vector fields on an open set
1
INDIVIDUAL TEST S.-T YAU COLLEGE MATH CONTESTS 2012
Geometry and Topology
Please solve 5 out of the following 6 problems, or highest scores of 5 problems will be counted.
