Particle Physics at Future Colliders
粒子加速器简介

• Radio Frequency Quadrupole (RFQ) • Drift tube Linac (DTL) • Cell-Coupled Drift Tube Linanc (CCDTL) • Side Coupled Linac (SCL) • Pulse rate 1.2 s
• Secondary particle beams: • From 1 GeV to ~ 15 GeV with ~ 106 particles • Protons, Electrons, Muons, Pions
Contents
• Why Accelerators and Colliders ? • The CERN Accelerator Complex • Cycling the Accelerators & Satisfying
Users • The Main Ingredients of an Accelerator • A brief word on the Future
is used to produce secondary particles
All energy will be available for particle production
Basics of Accelerator Physics and
Rende Steerenberg, BE-OP
Technology
Basics of Accelerator Physics and
Particle Physics

Matter constituents and force carriers
(1994 summary from the Contemporary Physics Education Project at LBNL)
And top is very very heavy !!!
Late 1920’s - early 1930’s: Dirac, Heisenberg, Pauli, & others Brief History of the Standard Model extend Maxwell’s theory of EM to include Special Relativity & QM (QED) - but it only works to lowest order! 1933: Fermi introduces 1st theory of weak interactions, analogous to QED, to explain b decay. 1935: Yukawa predicts the pion as carrier of a new, strong force to explain recently observed hadronic resonances. 1937: muon is observed in cosmic rays 1938: heavy W as mediator of weak interactions? (Klein) 1947: pion is observed in cosmic rays 1949: Dyson, Feynman, Schwinger, and Tomonaga introduce renormalization into QED - most accurate theory to date! 1954: Yang and Mills develop Gauge Theories 1950’s - early 1960’s: more than 100 hadronic “resonances” have been observed ! 1962 two neutrinos! 1964: Gell-Mann & Zweig propose a scheme whereby resonances
行星哥斯拉的荷电粒子炮原理

行星哥斯拉的荷电粒子炮原理行星哥斯拉的荷电粒子炮是一种强大的能量武器,它通过释放荷电粒子束来摧毁它的目标。
这项技术基于粒子物理学和高能物理学的原理,它的工作原理可以通过以下几个方面来解释。
首先,荷电粒子炮的核心部件是一个粒子加速器。
这个加速器使用强大的电磁场来加速带电粒子,通常是电子或质子,到非常高的速度。
这样的粒子加速器可以是线性加速器或环形加速器,通过不断的加速和轨道调节,粒子的能量就可以被增强到足够高的水平。
其次,经过粒子加速器加速的带电粒子被注入到一个大型储存环中。
这个储存环通常是一个环形真空腔,可以保持粒子在一个稳定的轨道上运动。
在这个储存环中,带电粒子被保持在稳定的状态,并且继续加速,将它们的能量增加到更高的水平。
接下来,一旦带电粒子的能量达到设定的水平,它们将被释放出来,形成一个荷电粒子束。
为了实现这一步骤,一个开关装置被用来打开储存环并且允许粒子束通过。
这个装置通常采用高强度电磁场或者激光脉冲来进行控制,确保粒子束能够准确地发射。
最后,荷电粒子束被聚焦和定向到行星哥斯拉的目标上。
为了实现这一点,粒子束经过一系列的聚焦和定向装置,以确保它们的能量可以准确地传递到目标上。
这些装置通常包括电磁透镜和磁铁,通过改变粒子的轨道来控制粒子束的传输方向和强度。
总的来说,行星哥斯拉的荷电粒子炮利用粒子加速器将带电粒子加速到非常高的能量水平,然后通过释放和聚焦这些粒子来形成一个强大的粒子束,从而摧毁目标。
这种技术结合了现代粒子物理学和高能物理学的原理,以及粒子加速器和聚焦技术的应用。
参考文献:1. Bane, K. L. F., & Chattopadhyay, S. (Eds.). (2013). Particle accelerators, colliders, and the story of high energy physics: charm, strange beauty. Springer Science & Business Media.2. Wilson, E. J. N. (2016). An introduction to particle accelerators. Oxford University Press.3. Wiedemann, H. (2015). Particle accelerator physics. Springer.4. Cottingham, W. N., & Greenwood, D. A. (2007). An introduction to nuclear physics. Cambridge University Press.。
Electron-Positron colliders

a r X i v :h e p -e x /0111070v 1 22 N o v 2001ELECTRON-POSITRON-COLLIDERSR.-D.HEUERInstitut f¨u r Experimentalphysik,Universit¨a t Hamburg,Luruper Chaussee 149,22761Hamburg GermanyE-mail:rolf-dieter.heuer@desy.deAn electron-positron linear collider in the energy range between 500and 1000GeV is of crucial importance to precisely test the Standard Model and to explore the physics beyond it.The physics program is complementary to that of the Large Hadron Collider.Some of the main physics goals and the expected accuracies of the anticipated measurements at such a linear collider are discussed.A short review of the different collider designs presently under study is given including possible upgrade paths to the multi-TeV region.Finally a framework is presented within which the realisation of such a project could be achieved as a global international project.1IntroductionA coherent picture of matter and forces has emerged in the past decades through in-tensive theoretical and experimental studies.It is adequately described by the Standard Model of particle physics.In the last few years many aspects of the model have been stringently tested,some to the per-mille level,with e +e −,ep and p ¯p machines making com-plementary contributions,especially to the determination of the electroweak bining the results with neutrino scatter-ing data and low energy measurements,the experimental analysis is in excellent concor-dance with the electroweak part of the Stan-dard Model.Also the predictions of QCD have been thoroughly tested,examples being precise measurements of the strong coupling αs and probing the proton structure to the shortest possible distances.Despite these great successes there are many gaps in our understanding.The clearest one is the present lack of any direct evidence for the dynamics of electroweak symmetry breaking and the generation of the masses of gauge bosons and fermions.The Higgs mechanism which generates the masses of the fundamental particles in the Standard Model,has not been experimentally estab-lished though the indirect evidence from pre-cision measurements is very strong.Even ifsuccessfully completed,the Standard Model does not provide a comprehensive theory of matter.There is no explanation for the wide range of masses of the fermions,the grand unification between the two gauge theories,electroweak and QCD,is not realised and gravity is not incorporated at the quantum level.Several alternative scenarios have been de-veloped for the physics which may emerge beyond the Standard Model as energies are increased.The Supersymmetric extension of the Standard Model provides a stable bridge from the presently explored energy scales up to the grand unification scale.Alternatively,new strong interactions give rise to strong forces between W bosons at high energies.Quite general arguments suggest that such new phenomena must appear below a scale of approximately 3TeV.Extra space dimen-sions which alter the high energy behaviour in such a way that the energy scale of gravity is in the same order as the electroweak scale are another proposed alternative.There are two ways of exploring the new scales,through attaining the highest possible energy in a hadron collider and through high precision measurements at the energy fron-tier of lepton colliders.This article is based on the results ofmany workshops on physics and detector studies for linear colliders.Much more can be found in the respective publications1,2,3,4 and on the different Web sites5,6,7,8.Many people have contributed to these studies and the references to their work can be found in the documents quoted above.2Complementarity of Lepton and Hadron MachinesIt is easier to accelerate protons to very high energies than leptons,but the detailed colli-sion process cannot be well controlled or se-lected.Electron-positron colliders offer a well√defined initial state.The collision energying generation of colliders.The physics case for such a machine will depend on the results from the LHC and the linear collider in the sub-TeV range.3Selected Physics TopicsIn this chapter,some of the main physics top-ics to be studied at a linear collider will bediscussed.Emphasis is given to the study of the Higgs mechanism in the Standard Model,the measurements of properties of su-persymmetric particles,and precision tests of the electroweak theory.More details about these topics as well as information about the numerous topics not presented here can be found in the physics books published in the studies of the physicspotential offuture lin-ear colliders 1,2,3,4.3.1Standard Model Higgs BosonThe main task of a linear electron-positron collider will be to establish experimentally the Higgs mechanism as the mechanism for generating the masses of fundamental parti-cles:•The Higgs boson must be discovered.•The couplings of the Higgs boson to gauge bosons and to fermions must be proven to increase with their masses.•The Higgs potential which generates the non-zero field in the vacuum must be reconstructed by determining the Higgs self-coupling.•The quantum numbers (J P C =0++)must be confirmed.The main production mechanisms for Higgs bosons in e +e −collisions are Higgs-strahlung e +e −→HZ and WW-fusion e +e −→νe ¯νe H ,and the corresponding cross-sections as a function of M H are depicted in figure 2for three different centre of mass en-ergies.With an integrated luminosity of 500Figure 2.The Higgs-strahlung and WW fusion pro-duction cross-sections as a function of M H for differ-ent√sof about 800GeV;for 1000fb −1an accuracyof 6%can be expected.The Higgs boson quantum numbers can be determined through the rise of the cross sec-tion close to the production threshold and through the angular distributions of the H and Z bosons in the continuum.Recoil Mass [GeV ]N u m b e r o f E v e n t s / 1.5 G e VFigure 3.The µ+µ−recoil mass distribution in theprocess e +e −→HZ →µ+µ−for M H =120GeV,500fb −1at√2of the self potential of the Higgs field V =λ(φ2−14λH4.The trilinearHiggs coupling λHHH =6λv can be mea-sured directly in the double Higgs-strahlung process e +e −→HHZ →q ¯q b ¯bb ¯b .The fi-nal state contains six partons resulting in a rather complicated experimental signature with six jets,a challenging task calling for ex-cellent granularity of the tracking device and the calorimeter 9.Despite the low cross sec-tion of the order of 0.2fb for M H =120GeV at√s =500GeV with an integrated luminosity of 1ab −1as shown in figure 6.Measurements of Higgs boson properties and their anticipated accuracies are sum-marised in table 1.In summary,the Higgs mechanism can be established in an unambiguous way at a high luminosity electron-positron collider with a centre-of-mass energy up to around one TeV as the mechanism responsible for the sponta-neous symmetry breaking of the electroweak interactions.3.2Supersymmetric ParticlesSupersymmetry (SUSY)is considered the most attractive extension of the Standardg c /g c (SM)g b /g b (S M )0.80.850.90.9511.051.11.151.2Figure 5.Higgs coupling determination:The con-tours for g b vs.g c for a 120GeV Higgs boson normalised to their Standard Model expectations as measured with 500fb −1.Model,which cannot be the ultimate the-ory for many reasons.The most impor-tant feature of SUSY is that it can explain the hierarchy between the electroweak scale of ≈100GeV,responsible for the W and Z masses,and the Planck scale M P l ≃1019GeV.When embedded in a grand-unified the-ory,it makes a very precise prediction of the electroweak mixing angle sin 2θW in excellent concordance with the precision electroweak measurement.In the following,only the min-imal supersymmetric extension to the Stan-dard Model (MSSM)will be considered and measurements of the properties of the super-symmetric particles will be discussed.Stud-ies of the supersymmetric Higgs sector can be found elsewhere 1,2,3,4.In addition to the particles of the Stan-dard Model,the MSSM contains their su-persymmetric partners:sleptons ˜l ±,˜νl (l =e,µ,τ),squarks ˜q ,and gauginos ˜g ,˜χ±,˜χ0.In the MSSM the multiplicative quantum num-ber R-parity is conserved,R p =+1for par-10012014016018000.20.10.3M H [GeV ]SM Double Higgs-strahlung: e + e - → ZHH σ [fb ]√s = 800 GeV√s = 500 GeVFigure 6.The cross-section for doubleHiggs-strahlung in the Standard Model at√120GeVmass 0.05%spin yes CPyes6%g HZZ 1%g HW W 2%g Hbb 2%g Hcc 10%g Hττ5%g Htt 6%λHHH∼30%ticles and R p =−1for sparticles.Spar-ticles are therefore produced in pairs and they eventually decay into the lightest spar-ticle which has to be stable.As an example,smuons are produced and decay through theprocess e +e −→˜µ+˜µ−→µ+µ−χ01χ01with χ01as the lightest sparticle being stable and,therefore,escaping detection.The mass scale of sparticles is only vaguely known.In most scenarios some spar-ticles,in particular charginos and neutrali-nos,are expected to lie in the energy region accessible by the next generation of e +e −200400600800Figure 7.Examples of mass spectra in mSUGRA,GMSB and AMSB models.colliders alsosupported bythe recentmea-surement of (g −2)µ10.Examples of massspectra for three SUSY breaking mechanisms (mSUGRA,GMSB,AMSB)are given in fig-ure 7.The most fundamental problem of super-symmetric theories is how SUSY is broken and in which way this breaking is communi-cated to the particles.Several scenarios have been proposed in which the mass spectra are generally quite different as illustrated in fig-ure 7.High precision measurements of the particle properties are therefore expected to distinguish between some of these scenarios.The study and exploration of Supersymmetry will proceed in the following steps:•Reconstruction of the kinematically ac-cessible spectrum of sparticles and the measurement of their properties,masses and quantum numbers•Extraction of the basic low-energy pa-rameters such as mass parameters,cou-plings,and mixings•Analysis of the breaking mechanism and reconstruction of the underlying theory.While it is unlikely that the complete spectrum of sparticles will be accessible at acollider with√Figure 9.Cross section near threshold for the processe +e −→˜χ+1˜χ−1,10fb−1per point.approach,the measured electroweak scaleSUSY parameters are extrapolated to high energies using these RGE’s.Due to the high precision of the measured input variables,only possible at the linear collider,an accurate test can be performed at which energy scale certain parameters be-come equal.Most interesting,the assump-tion of grand unification of forces requires the gaugino mass parameters M 1,M 2,M 3to meet at the GUT scale (figure 10(left)).Different SUSY breaking mechanisms predict different unification patterns of the sfermion mass parameters at high energy.With the high accuracy of the linear collider measure-ments these models can be distinguished as shown in figure 10for the case of mSUGRA (middle)and GMSB (right).In summary,the high precision studies of supersymmetric particles and their properties can open a window to energy scales far above the scales reachable with future accelerators,possibly towards the Planck scale where grav-ity becomes important.3.3Precision MeasurementsThe primary goal of precision measurements of gauge boson properties is to establish the non-abelian nature of electroweak interac-tions.The gauge symmetries of the Stan-dard Model determine the form and the strength of the self-interactions of the elec-troweak bosons,the triple couplings W W γand W W Z and the quartic couplings.Devi-ations from the Standard Model expectations for these couplings could be expected in sev-eral scenarios,for example in models where there exists no light Higgs boson and where the W and Z bosons are generated dynam-ically and interact strongly at high scales.Also for the extrapolation of couplings to high scales to test theories of grand unifi-cation such high precision measurements are mandatory.For the study of the couplings between gauge bosons the best precision is reached at the highest possible centre of mass energies.These couplings are especially sen-sitive to models of strong electroweak sym-metry breaking.W bosons are produced either in pairs,e +e −→W +W −or singly,e +e −→W eνwith both processes being sensitive to the triple gauge couplings.In general the total errors estimated on the anomalous couplings are in the range of few ×10−4.Figure 11com-pares the precision obtainable for ∆κγand ∆λγat different machines.The measurements at a linear collider are sensitive to strong symmetry breaking be-yond Λof the order of 5TeV,to be com-pared with the electroweak symmetry break-ing scale ΛEW SB =4πv ≈3TeV.One of the most sensitive quantities to loop corrections from the Higgs boson is the effective weak mixing angle in Z boson de-cays.By operating the collider at ener-gies close to the Z -pole with high luminos-ity (GigaZ)to collect at least 109Z bosons in particular the accuracy of the measure-Figure 10.Extrapolation of SUSY parameters measured at the electroweak scale to high energies.10-410-310-2∆κγLEP TEV LHCTESLA TESLA 50080010-410-310-2∆λγLEP TEV LHCTESLA TESLA500800Figure parison of constraints on the anomalous couplings ∆κγand ∆λγat different machinesment of sin 2θleff can be improved by one or-der of magnitude wrt.the precision obtained today 11.With both electron and positronbeams longitudinally polarised,sin 2θleff can be determined most accurately by measur-ing the left-right asymmetry A LR =A e =2v e a e /(v 2e +a 2e )with v e (a e )being the vec-tor (axialvector)couplings of the Z boson tothe electron and v e /a e =1-4sin 2θleff for pure Z exchange.Particularly demanding is the precision of 2×10−4with which the po-larisation needs to be known to match the statistical accuracy.An error in the weakmixing angle of ∆sin 2θleff =0.000013can be expected.Together with an improved de-termination of the mass of the W boson toa precision of some 6MeV through a scan of the W W production threshold and with the measurements obtained at high energy run-ning of the collider this will allow many high precision tests of the Standard Model at the loop level.As an example,figure 12shows the variation of the fit χ2to the electroweak measurements as a function of M H for the present data and for the data expected at a linear collider.The mass of the Higgs bo-son can indirectly be constraint at a level of 5%.Comparing this prediction with the di-rect measurement of M H consistency tests of the Standard Model can be performed at the quantum level or to measure free parameters in extensions of the Standard Model.This is5101520101032000LCm hχ2Figure 12.∆χ2as a function of the Higgs boson mass for the electroweak precision data today (2000)and after GigaZ running (LC).of particular importance if M H >200GeV in contradiction to the current electroweak mea-surements.In summary,there is strong evidence for new phenomena at the TeV energy scale.Only the precision exploration at the linear collider will allow,together with the results obtained at the Large Hadron Collider,the understanding of the underlying physics and will open a new window beyond the centre-of-mass energies reachable.Whatever sce-nario is realized in nature,the linear collider will add crucial information beyond the LHC.There is global consensus in the high energy physics community that the next accelera-tor based project needs to be an electron-positron linear collider with a centre-of-mass energy of at least 500GeV.4Electron-Positron Linear CollidersThe feasibility of a linear collider has been successfully demonstrated by the operationof the SLAC Linear Collider,SLC.How-ever,aiming at centre-of-mass energies at the TeV scale with luminosities of the order of 1034cm −2s −1requires at least two orders of magnitude higher beam power and two orders of magnitude smaller beam sizes at the inter-action point.Over the past decade,several groups worldwide have been pursuing differ-ent linear collider designs for the centre-of-mass energy range up to around one TeV as well as for the multi-TeV range.Excel-lent progress has been achieved at various test facilities worldwide in international col-laborations on crucial aspects of the collider designs.At the Accelerator Test Facility at KEK 12,emittances within a factor two of the damping ring design have been achieved.At the Final Focus Test Beam at SLAC 13de-magnification of the beams has been proven;the measured spot sizes are well in agreement with the theoretically expected values.The commissioning and operation of the TESLA Test Facility at DESY 14has demonstrated the feasibility of the TESLA technology.In the following,a short review of the different approaches is given.4.1TeV rangeThree design studies are presently pursued:JLC 15,NLC 16and TESLA 17,centred around KEK,SLAC and DESY,respectively.Details about the design,the status of de-velopment and the individual test facilities can be found in the above quoted references as well as in the status reports presented at LCWS200018,19,20.A comprehensive sum-mary of the present status can be found in the Snowmass Accelerator R&D Report 21,here only a short discussion of the main features and differences of the three approaches will be given with emphasis on luminosity and en-ergy reach.One key parameter for performing the physics program at a collider is the centre-of-mass energy achievable.The energy reachof a collider with a given linac length and a certain cavityfilling factor is determined by the gradient achievable with the cavity tech-nology chosen.For normalconducting cavi-ties the maximum achievable gradient scales roughly proportional to the RF frequency used,for superconducting Niobium cavities, the fundamental limit today is around55 MV/m.The second key parameter for the physicsprogram is the luminosity L,given byL=n b N2e f rep(σ∗x+σ∗y)2.Choos-ing aflat beam size(σ∗x≫σ∗y)at the inter-action point,δE becomes independent of the vertical beam size and the luminosity can be increased by reducingσ∗y as much as possi-ble.Sinceσ∗y∝sn b N e f rep=ηP AC is obtained from themains power P AC with an efficiency η.Equation(1)can then be rewritten asL∝ηP AC s ǫy(2)High luminosity therefore requires high ef-ficiencyηand high beam quality with low emittanceǫy and low emittance dilution ∆ǫ/ǫ∝f6RF,which is largely determined by the RF frequency f RF of the chosen technol-ogy.The fundamental difference between the three designs is the choice of technology for the accelerating structures.The design of NLC is based on normalconducting cavities using f RF of11.4GHz(X-band),for JLC two options,X-band or C-band(5.7GHz)are pursued.The TESLA concept,developed by the TESLA collaboration,is using supercon-ducting cavities(1.3GHz).As an example for a linear collider facility,figure13shows the schematic layout of TESLA.Figure13.Schematic layout of TESLATable2compares some key parameters for the different technologies at√Figure 14.Evolution of superconducting cavity per-formance.The average gradient achieved with TESLA 9-cell cavities produced in industry (first test,no additional processing)is shown as dots.with N b bunches,the time ∆T b between bunches within a train which allows head on crossing of the bunches for TESLA but requires a crossing angle for the other de-signs.The design luminosity L ,beam power P beam and the required mains power P AC il-lustrate that for a given mains power the su-perconducting technology delivers higher lu-minosity.On the other hand the lower gradi-ent G acc requires a longer linac for the samecentre-of-massenergy reach.As can be seen from table 2the X-band machines call for a beam loaded (unloaded)gradient of some 50(70)MV/m for√s =500GeV,a gradient which is mean-while routinely achieved for cavities fabri-cated in industry as illustrated in figure 14.Table 2also contains the presently planned length of the facilities 17,16,22,23.AnFigure 15.Excitation curves of three electropolished single-cell cavities.Gradients well above 35MV/m are reached.upgrade in energy up to around one TeV seems possible for all designs.In the NLC case,more cavities would be installed within the existing tunnel,in the JLC case,the tunnel length would have to be increased to house more cavities.In the TESLA case,a gradient of around 35MV/m is neededto reach√Table parison of some crucial parameters at 500GeV for the different technologies under study,see text for details.NLCJLC-C51502820190337 1.4head on angle 20.7σ∗x/y [nm ]245/2.7318/4.3δE [%]4.73.93.42.64P beam [MW ]13.212.6P AC (linacs )[MW ]13222023.550.23316s of 3TeV,usinghigh frequency (30GHz)normalcon-ducting structures operating at very high ac-celerating fields (150MV/m).The present design calls for bunch separations of .67ns,a vertical spotsize of 1nm and beamstrahlung δE of 30%.For this promising concept a new test facility is under construction at CERN which should allow tests with full gradient starting in 2005.5RealisationThe new generation of high energy colliders most likely exceeds the resources of a coun-try or even a region.There is general consen-sus that the realisation has to be done in an international,interregional framework.One such framework,the so called Global Accel-erator Network (GAN),has been proposed to ICFA in March 2000.A short discussion of the principle considerations will be presented here,more details can be found in ref.25.The GAN is a global collaboration of lab-oratories and institutes in order to design,construct,commission,operate and main-tain a large accelerator facility.The model is based on the experience of large experi-mental collaborations,particularly in particle physics.Some key elements are listed below:•it is not an international permanent in-stitution,but an international project of limited duration;•the facility would be the common prop-erty of the participating countries;•there are well defined roles and obliga-tions of all partners;•partners contribute through components or subsystems;•design,construction and testing of com-ponents is done in participating institu-tions;•maintenance and running of the accel-erator would be done to a large extent from the participating institutions.The GAN would make best use of world-wide competence,ideas and resources,create a visible presence of activities in all partici-pating countries and would,hopefully,make the site selection less controversial.study general considerations of implementing a GAN and to study the technical considera-tions and influence on the design and cost of the accelerator.The reports of these working groups can be found on the web26.Their overall conclusion is that a GAN can be a fea-sible way to build and operate a new global accelerator,although many details still need to be clarified.6SummaryThere is global consensus about the next ac-celerator based project in particle physics.It has to be an electron-positron linear collider with an initial energy reach of some500GeV with the potential of an upgrade in centre-of-mass energy.The physics case is excellent, only a few highlights could be presented here. There is also global consensus that concur-rent operation with LHC is needed and fruit-ful.Therefore,a timely realisation is manda-tory.The technical realisation of a linear col-lider is now feasible,several technologies are either ripe or will be ripe soon.A fast consen-sus in the community about the technology is as a global project with the highest possible luminosity and a clear upgrade potential be-yond500GeV.AcknowledgmentsThe author would like to express his grati-tude to all people who have contributed to the studies of future electron-positron linear colliders from the machine design to physics and detector studies.Special thanks go to the organisers and their team for a very well or-ganised,inspiring conference as well as for the competent technical help in preparing this presentation.References1.J.A.Aguilar-Saavedra et al,TESLATechnical Design Report,Part III,Physics at an e+e−Linear Collider,DESY2001-011,ECFA2001-209,hep-ph/0106315.2.T.Abe et al,Linear Collider Physics Re-source Book for Snowmass2001,BNL-52627,CLNS01/1729,FERMILAB-Pub-01/058-E,LBNL-47813,SLAC-R-570,UCRL-ID-143810-DR,LC-REV-2001-074-US,hep-ex/0106055-583.K.Abe et al,Particle Physics Exper-iments at JLC,KEK-Report2001-11, hep-ph/0109166.4.Proceedings of LCWS,Physics and Ex-periments with Future Linear Colliders, eds A.Para,H.E.Fisk,(AIP Conf.Proc.,Vol578,2001).5.Worldwide Study of the Physics and De-tectors for Future e+e−Colliders/lc/6.ACFA Joint Linear Collider Physics andDetector Working Grouphttp://acfahep.kek.jp/7.2nd Joint ECFA/DESY Studyon Physics and Detectors for a Linear Electron-Positron Colliderhttp://www.desy.de/conferences/ecfa-desy-lc98.html8.A Study of the Physics and Detectors forFuture Linear e+e−Colliders:American Activities/lc/ameri-ca.html9.G.Alexander et al,TESLA TechnicalDesign Report,Part IV,A Detector for TESLA,DESY2001-011,ECFA2001-209.10.H.N.Brown et al.[Muon g-2Collabo-ration],Phys.Rev.Lett.86(2001)222711.J.Drees,these proceedings12.E.Hinode et al,eds.,KEK Internal95-4,1995,eds J.Urakawa and M.Yoshioka, Proceedings of the SLAC/KEK Linear Collider Workshop on Damping Ring, KEK92-6,199213.The FFTB Collaboration:BINP(Novosibirsk/Protvino),DESY, FNAL,KEK,LAL(Orsay),MPI Mu-nich,Rochester,and SLAC14.Proposal for a TESLA Test Facility,DESY TESLA-93-01,199215.KEK-Report97-1,1997.16.Zeroth Order Design Report for theNext Linear Collider,SLAC Report474,1996.2001Report on the Next Linear Collider,Fermilab-Conf-01-075-E,LBNL-47935,SLAC-R-571,UCRL-ID-14407717.J.Andruszkow et al,TESLA TechnicalDesign Report,Part II,The Accelerator, DESY2001-011,ECFA2001-20918.O.Napoly,TESLA Linear Collider:Sta-tus Report,in ref419.T.O.Raubenheimer,Progress in theNext Linear Collider Design,in ref4 20.Y.H.Chin et al Status of JLC Accelera-tor Development,in ref421.A.Chao et al,2001Snowmass Accelera-tor R&D Report,http://www.hep.anl.gov/pvs/dpb/Snowmass.pdf22.Y.H.Chin,private communication23.H.Matsumoto,T.Shintake,private com-munication24.I.Wilson,A Multi-TeV Compact e+e−Linear Collider,in ref425.F.Richard et al,TESLA Technical De-sign Report,Part I,Executive Summary, DESY2001-011,ECFA2001-209,hep-ph/0106314.26./directorate/icfa/icfa reports.html。
等离子体透镜

FOR FUTURE ee- high-energy linear colliders, one
tions on the focusing process through a thin lens. The results show excellent agreement with the theoretical prediction. We then turn to the discussion of a thick plasma lens based on the same physical concept. The fact that the beam particles execute substantial transverse motion during interaction with the thick plasma lens forbids simple analytic description of the beam dynamics which is both nonlinear and convolutional. We therefore rely on computer simulations in this respect in Section V. Summary and discussion are given at the end. We discuss the advantages and disadvantages when one replaces the particle beam precursor by a beating laser beam.
科学故事的英语作文

科学故事的英语作文英文回答:Science is an exciting and ever-evolving field that constantly provides new insights into the world around us. From the smallest particles of matter to the vastness of the cosmos, science offers endless opportunities for exploration and discovery.One fascinating scientific story that captures my imagination is the tale of the discovery of the doublehelix structure of DNA. In the 1950s, scientists James Watson and Francis Crick were working on determining the molecular structure of DNA, which is the genetic material of all living organisms. They used X-ray crystallography to study DNA fibers, and with the help of Rosalind Franklin's X-ray diffraction patterns, they were able to deduce the double helix shape of the DNA molecule.This discovery was a groundbreaking moment in biology,as it provided a physical explanation for how genetic information is stored and transmitted. The double helix structure of DNA has also led to advancements in genetics, medicine, and biotechnology. For example, scientists have been able to develop DNA sequencing techniques that allow them to read the genetic code of organisms, and this information can be used for diagnostic purposes, genetic counseling, and personalized medicine.Another captivating scientific story is the discovery of the Higgs boson, which was predicted by the Standard Model of particle physics. In 2012, scientists at the Large Hadron Collider at CERN in Switzerland announced that they had observed a new particle that was consistent with the properties of the Higgs boson. The Higgs boson is responsible for giving other particles their mass, and its discovery was a major confirmation of the Standard Model.The discovery of the Higgs boson has opened up new avenues of research in particle physics, and it has also led to the development of new theories beyond the Standard Model. Scientists are now exploring the possibility ofsupersymmetry, which is a theory that predicts theexistence of new particles that have not yet been observed.These are just two examples of the many intriguing scientific stories that have shaped our understanding ofthe world. Science continues to push the boundaries of human knowledge, and it is sure to provide us with evenmore fascinating discoveries in the years to come.中文回答:科学是一个激动人心且不断发展的领域,它不断为我们周围的世界带来新的见解。
The Standard Model of Particle Physics

a r X i v :h e p -p h /9812285v 1 8 D e c 1998The Standard Model of Particle PhysicsMary K.Gaillard 1,Paul D.Grannis 2,and Frank J.Sciulli 31University of California,Berkeley,2State University of New York,Stony Brook,3Columbia UniversityParticle physics has evolved a coherent model that characterizes forces and particles at the mostelementary level.This Standard Model,built from many theoretical and experimental studies,isin excellent accord with almost all current data.However,there are many hints that it is but anapproximation to a yet more fundamental theory.We trace the development of the Standard Modeland indicate the reasons for believing that it is incomplete.Nov.20,1998(To be published in Reviews of Modern Physics)I.INTRODUCTION:A BIRD’S EYE VIEW OF THE STANDARD MODEL Over the past three decades a compelling case has emerged for the now widely accepted Standard Model of elementary particles and forces.A ‘Standard Model’is a theoretical framework built from observation that predicts and correlates new data.The Mendeleev table of elements was an early example in chemistry;from the periodic table one could predict the properties of many hitherto unstudied elements and compounds.Nonrelativistic quantum theory is another Standard Model that has correlated the results of countless experiments.Like its precursors in other fields,the Standard Model (SM)of particle physics has been enormously successful in predicting a wide range of phenomena.And,just as ordinary quantum mechanics fails in the relativistic limit,we do not expect the SM to be valid at arbitrarily short distances.However its remarkable success strongly suggests that the SM will remain an excellent approximation to nature at distance scales as small as 10−18m.In the early 1960’s particle physicists described nature in terms of four distinct forces,characterized by widely different ranges and strengths as measured at a typical energy scale of 1GeV.The strong nuclear force has a range of about a fermi or 10−15m.The weak force responsible for radioactive decay,with a range of 10−17m,is about 10−5times weaker at low energy.The electromagnetic force that governs much of macroscopic physics has infinite range and strength determined by the finestructure constant,α≈10−2.The fourth force,gravity,also has infinite range and a low energy coupling (about 10−38)too weak to be observable in laboratory experiments.The achievement of the SM was the elaboration of a unified description of the strong,weak and electromagnetic forces in the language of quantum gauge field theories.Moreover,the SM combines the weak and electromagnetic forces in a single electroweak gauge theory,reminiscent of Maxwell’s unification of the seemingly distinct forces of electricity and magnetism.By mid-century,the electromagnetic force was well understood as a renormalizable quantum field theory (QFT)known as quantum electrodynamics or QED,described in the preceeding article.‘Renormalizable’means that once a few parameters are determined by a limited set of measurements,the quantitative features of interactions among charged particles and photons can be calculated to arbitrary accuracy as a perturbative expansion in the fine structure constant.QED has been tested over an energy range from 10−16eV to tens of GeV,i.e.distances ranging from 108km to 10−2fm.In contrast,the nuclear force was characterized by a coupling strength that precluded a perturbativeexpansion.Moreover,couplings involving higher spin states(resonances),that appeared to be onthe same footing as nucleons and pions,could not be described by a renormalizable theory,nor couldthe weak interactions that were attributed to the direct coupling of four fermions to one another.In the ensuing years the search for renormalizable theories of strong and weak interactions,coupledwith experimental discoveries and attempts to interpret available data,led to the formulation ofthe SM,which has been experimentally verified to a high degree of accuracy over a broad range ofenergy and processes.The SM is characterized in part by the spectrum of elementaryfields shown in Table I.The matterfields are fermions and their anti-particles,with half a unit of intrinsic angular momentum,or spin.There are three families of fermionfields that are identical in every attribute except their masses.Thefirst family includes the up(u)and down(d)quarks that are the constituents of nucleons aswell as pions and other mesons responsible for nuclear binding.It also contains the electron and theneutrino emitted with a positron in nuclearβ-decay.The quarks of the other families are constituentsof heavier short-lived particles;they and their companion charged leptons rapidly decay via the weakforce to the quarks and leptons of thefirst family.The spin-1gauge bosons mediate interactions among fermions.In QED,interactions among elec-trically charged particles are due to the exchange of quanta of the electromagneticfield called photons(γ).The fact that theγis massless accounts for the long range of the electromagnetic force.Thestrong force,quantum chromodynamics or QCD,is mediated by the exchange of massless gluons(g)between quarks that carry a quantum number called color.In contrast to the electrically neutralphoton,gluons(the quanta of the‘chromo-magnetic’field)possess color charge and hence couple toone another.As a consequence,the color force between two colored particles increases in strengthwith increasing distance.Thus quarks and gluons cannot appear as free particles,but exist onlyinside composite particles,called hadrons,with no net color charge.Nucleons are composed ofthree quarks of different colors,resulting in‘white’color-neutral states.Mesons contain quark andanti-quark pairs whose color charges cancel.Since a gluon inside a nucleon cannot escape its bound-aries,the nuclear force is mediated by color-neutral bound states,accounting for its short range,characterized by the Compton wavelength of the lightest of these:theπ-meson.The even shorter range of the weak force is associated with the Compton wave-lengths of thecharged W and neutral Z bosons that mediate it.Their couplings to the‘weak charges’of quarksand leptons are comparable in strength to the electromagnetic coupling.When the weak interactionis measured over distances much larger than its range,its effects are averaged over the measurementarea and hence suppressed in amplitude by a factor(E/M W,Z)2≈(E/100GeV)2,where E is the characteristic energy transfer in the measurement.Because the W particles carry electric charge theymust couple to theγ,implying a gauge theory that unites the weak and electromagnetic interactions,similar to QCD in that the gauge particles are self-coupled.In distinction toγ’s and gluons,W’scouple only to left-handed fermions(with spin oriented opposite to the direction of motion).The SM is further characterized by a high degree of symmetry.For example,one cannot performan experiment that would distinguish the color of the quarks involved.If the symmetries of theSM couplings were fully respected in nature,we would not distinguish an electron from a neutrinoor a proton from a neutron;their detectable differences are attributed to‘spontaneous’breakingof the symmetry.Just as the spherical symmetry of the earth is broken to a cylindrical symmetry by the earth’s magneticfield,afield permeating all space,called the Higgsfield,is invoked to explain the observation that the symmetries of the electroweak theory are broken to the residual gauge symmetry of QED.Particles that interact with the Higgsfield cannot propagate at the speed of light,and acquire masses,in analogy to the index of refraction that slows a photon traversing matter.Particles that do not interact with the Higgsfield—the photon,gluons and possibly neutrinos–remain massless.Fermion couplings to the Higgsfield not only determine their masses; they induce a misalignment of quark mass eigenstates with respect to the eigenstates of the weak charges,thereby allowing all fermions of heavy families to decay to lighter ones.These couplings provide the only mechanism within the SM that can account for the observed violation of CP,that is,invariance of the laws of nature under mirror reflection(parity P)and the interchange of particles with their anti-particles(charge conjugation C).The origin of the Higgsfield has not yet been determined.However our very understanding of the SM implies that physics associated with electroweak symmetry breaking(ESB)must become manifest at energies of present colliders or at the LHC under construction.There is strong reason, stemming from the quantum instability of scalar masses,to believe that this physics will point to modifications of the theory.One shortcoming of the SM is its failure to accommodate gravity,for which there is no renormalizable QFT because the quantum of the gravitationalfield has two units of spin.Recent theoretical progress suggests that quantum gravity can be formulated only in terms of extended objects like strings and membranes,with dimensions of order of the Planck length10−35m. Experiments probing higher energies and shorter distances may reveal clues connecting SM physics to gravity,and may shed light on other questions that it leaves unanswered.In the following we trace the steps that led to the formulation of the SM,describe the experiments that have confirmed it,and discuss some outstanding unresolved issues that suggest a more fundamental theory underlies the SM.II.THE PATH TO QCDThe invention of the bubble chamber permitted the observation of a rich spectroscopy of hadron states.Attempts at their classification using group theory,analogous to the introduction of isotopic spin as a classification scheme for nuclear states,culminated in the‘Eightfold Way’based on the group SU(3),in which particles are ordered by their‘flavor’quantum numbers:isotopic spin and strangeness.This scheme was spectacularly confirmed by the discovery at Brookhaven Laboratory (BNL)of theΩ−particle,with three units of strangeness,at the predicted mass.It was subsequently realized that the spectrum of the Eightfold Way could be understood if hadrons were composed of three types of quarks:u,d,and the strange quark s.However the quark model presented a dilemma: each quark was attributed one half unit of spin,but Fermi statistics precluded the existence of a state like theΩ−composed of three strange quarks with total spin3A combination of experimental observations and theoretical analyses in the1960’s led to anotherimportant conclusion:pions behave like the Goldstone bosons of a spontaneously broken symmetry,called chiral symmetry.Massless fermions have a conserved quantum number called chirality,equalto their helicity:+1(−1)for right(left)-handed fermions.The analysis of pion scattering lengths andweak decays into pions strongly suggested that chiral symmetry is explicitly broken only by quarkmasses,which in turn implied that the underlying theory describing strong interactions among quarksmust conserve quark helicity–just as QED conserves electron helicity.This further implied thatinteractions among quarks must be mediated by the exchange of spin-1particles.In the early1970’s,experimenters at the Stanford Linear Accelerator Center(SLAC)analyzed thedistributions in energy and angle of electrons scattered from nuclear targets in inelastic collisionswith momentum transfer Q2≈1GeV/c from the electron to the struck nucleon.The distributions they observed suggested that electrons interact via photon exchange with point-like objects calledpartons–electrically charged particles much smaller than nucleons.If the electrons were scatteredby an extended object,e.g.a strongly interacting nucleon with its electric charge spread out by acloud of pions,the cross section would drop rapidly for values of momentum transfer greater than theinverse radius of the charge distribution.Instead,the data showed a‘scale invariant’distribution:across section equal to the QED cross section up to a dimensionless function of kinematic variables,independent of the energy of the incident electron.Neutrino scattering experiments at CERN andFermilab(FNAL)yielded similar parison of electron and neutrino data allowed adetermination of the average squared electric charge of the partons in the nucleon,and the result wasconsistent with the interpretation that they are fractionally charged quarks.Subsequent experimentsat SLAC showed that,at center-of-mass energies above about two GeV,thefinal states in e+e−annihilation into hadrons have a two-jet configuration.The angular distribution of the jets withrespect to the beam,which depends on the spin of thefinal state particles,is similar to that of themuons in anµ+µ−final state,providing direct evidence for spin-1√where G F is the Fermi coupling constant,γµis a Dirac matrix and12fermions via the exchange of spinless particles.Both the chiral symmetry of thestrong interactions and the V−A nature of the weak interactions suggested that all forces except gravity are mediated by spin-1particles,like the photon.QED is renormalizable because gauge invariance,which gives conservation of electric charge,also ensures the cancellation of quantum corrections that would otherwise result in infinitely large amplitudes.Gauge invariance implies a massless gauge particle and hence a long-range force.Moreover the mediator of weak interactions must carry electric charge and thus couple to the photon,requiring its description within a Yang-Mills theory that is characterized by self-coupled gauge bosons.The important theoretical breakthrough of the early1970’s was the proof that Yang-Mills theories are renormalizable,and that renormalizability remains intact if gauge symmetry is spontaneously broken,that is,if the Lagrangian is gauge invariant,but the vacuum state and spectrum of particles are not.An example is a ferromagnet for which the lowest energy configuration has electron spins aligned;the direction of alignment spontaneously breaks the rotational invariance of the laws ofphysics.In QFT,the simplest way to induce spontaneous symmetry breaking is the Higgs mech-anism.A set of elementary scalarsφis introduced with a potential energy density function V(φ) that is minimized at a value<φ>=0and the vacuum energy is degenerate.For example,the gauge invariant potential for an electrically charged scalarfieldφ=|φ|e iθ,V(|φ|2)=−µ2|φ|2+λ|φ|4,(3)√λ=v,but is independent of the phaseθ.Nature’s choice forθhas its minimum atspontaneously breaks the gauge symmetry.Quantum excitations of|φ|about its vacuum value are massive Higgs scalars:m2H=2µ2=2λv2.Quantum excitations around the vacuum value ofθcost no energy and are massless,spinless particles called Goldstone bosons.They appear in the physical spectrum as the longitudinally polarized spin states of gauge bosons that acquire masses through their couplings to the Higgsfield.A gauge boson mass m is determined by its coupling g to theHiggsfield and the vacuum value v.Since gauge couplings are universal this also determines the√Fermi constant G for this toy model:m=gv/2,G/2|φ|=212F=246GeV,leaving three Goldstone bosons that are eaten by three massive vector bosons:W±and Z=cosθw W0−sinθw B0,while the photonγ=cosθw B0+sinθw W0remains massless.This theory predicted neutrino-induced neutral current(NC)interactions of the typeν+atom→ν+anything,mediated by Z exchange.The weak mixing angleθw governs the dependence of NC couplings on fermion helicity and electric charge, and their interaction rates are determined by the Fermi constant G Z F.The ratioρ=G Z F/G F= m2W/m2Z cos2θw,predicted to be1,is the only measured parameter of the SM that probes thesymmetry breaking mechanism.Once the value ofθw was determined in neutrino experiments,the√W and Z masses could be predicted:m2W=m2Z cos2θw=sin2θwπα/QUARKS:S=1LEPTONS:S=13m3m Q=0m quanta mu1u2u3(2–8)10−3e 5.11×10−4c1c2c3 1.0–1.6µ0.10566t1t2t3173.8±5.0τ 1.77705/3g′,where g1isfixed by requiring the same normalization for all fermion currents.Their measured values at low energy satisfy g3>g2>g1.Like g3,the coupling g2decreases with increasing energy,but more slowly because there are fewer gauge bosons contributing.As in QED,the U(1)coupling increases with energy.Vacuum polarization effects calculated using the particle content of the SM show that the three coupling constants are very nearly equal at an energy scale around1016GeV,providing a tantalizing hint of a more highly symmetric theory,embedding the SM interactions into a single force.Particle masses also depend on energy;the b andτmasses become equal at a similar scale,suggesting a possibility of quark and lepton unification as different charge states of a singlefield.V.BRIEF SUMMARY OF THE STANDARD MODEL ELEMENTSThe SM contains the set of elementary particles shown in Table I.The forces operative in the particle domain are the strong(QCD)interaction responsive to particles carrying color,and the two pieces of the electroweak interaction responsive to particles carrying weak isospin and hypercharge. The quarks come in three experimentally indistinguishable colors and there are eight colored gluons. All quarks and leptons,and theγ,W and Z bosons,carry weak isospin.In the strict view of the SM,there are no right-handed neutrinos or left-handed anti-neutrinos.As a consequence the simple Higgs mechanism described in section IV cannot generate neutrino masses,which are posited to be zero.In addition,the SM provides the quark mixing matrix which gives the transformation from the basis of the strong interaction charge−1Finding the constituents of the SM spanned thefirst century of the APS,starting with the discovery by Thomson of the electron in1897.Pauli in1930postulated the existence of the neutrino as the agent of missing energy and angular momentum inβ-decay;only in1953was the neutrino found in experiments at reactors.The muon was unexpectedly added from cosmic ray searches for the Yukawa particle in1936;in1962its companion neutrino was found in the decays of the pion.The Eightfold Way classification of the hadrons in1961suggested the possible existence of the three lightest quarks(u,d and s),though their physical reality was then regarded as doubtful.The observation of substructure of the proton,and the1974observation of the J/ψmeson interpreted as a cp collider in1983was a dramatic confirmation of this theory.The gluon which mediates the color force QCD wasfirst demonstrated in the e+e−collider at DESY in Hamburg.The minimal version of the SM,with no right-handed neutrinos and the simplest possible ESB mechanism,has19arbitrary parameters:9fermion masses;3angles and one phase that specify the quark mixing matrix;3gauge coupling constants;2parameters to specify the Higgs potential; and an additional phaseθthat characterizes the QCD vacuum state.The number of parameters is larger if the ESB mechanism is more complicated or if there are right-handed neutrinos.Aside from constraints imposed by renormalizability,the spectrum of elementary particles is also arbitrary.As discussed in Section VII,this high degree of arbitrariness suggests that a more fundamental theory underlies the SM.VI.EXPERIMENTAL ESTABLISHMENT OF THE STANDARD MODELThe current picture of particles and interactions has been shaped and tested by three decades of experimental studies at laboratories around the world.We briefly summarize here some typical and landmark results.FIG.1.The proton structure function(F2)versus Q2atfixed x,measured with incident electrons or muons,showing scale invariance at larger x and substantial dependence on Q2as x becomes small.The data are taken from the HERA ep collider experiments H1and ZEUS,as well as the muon scattering experiments BCDMS and NMC at CERN and E665at FNAL.A.Establishing QCD1.Deep inelastic scatteringPioneering experiments at SLAC in the late1960’s directed high energy electrons on proton and nuclear targets.The deep inelastic scattering(DIS)process results in a deflected electron and a hadronic recoil system from the initial baryon.The scattering occurs through the exchange of a photon coupled to the electric charges of the participants.DIS experiments were the spiritual descendents of Rutherford’s scattering ofαparticles by gold atoms and,as with the earlier experi-ment,showed the existence of the target’s substructure.Lorentz and gauge invariance restrict the matrix element representing the hadronic part of the interaction to two terms,each multiplied by phenomenological form factors or structure functions.These in principle depend on the two inde-pendent kinematic variables;the momentum transfer carried by the photon(Q2)and energy loss by the electron(ν).The experiments showed that the structure functions were,to good approximation, independent of Q2forfixed values of x=Q2/2Mν.This‘scaling’result was interpreted as evi-dence that the proton contains sub-elements,originally called partons.The DIS scattering occurs as the elastic scatter of the beam electron with one of the partons.The original and subsequent experiments established that the struck partons carry the fractional electric charges and half-integer spins dictated by the quark model.Furthermore,the experiments demonstrated that three such partons(valence quarks)provide the nucleon with its quantum numbers.The variable x represents the fraction of the target nucleon’s momentum carried by the struck parton,viewed in a Lorentz frame where the proton is relativistic.The DIS experiments further showed that the charged partons (quarks)carry only about half of the proton momentum,giving indirect evidence for an electrically neutral partonic gluon.1011010101010FIG.2.The quark and gluon momentum densities in the proton versus x for Q 2=20GeV 2.The integrated values of each component density gives the fraction of the proton momentum carried by that component.The valence u and d quarks carry the quantum numbers of the proton.The large number of quarks at small x arise from a ‘sea’of quark-antiquark pairs.The quark densities are from a phenomenological fit (the CTEQ collaboration)to data from many sources;the gluon density bands are the one standard deviation bounds to QCD fits to ZEUS data (low x )and muon scattering data (higher x ).Further DIS investigations using electrons,muons,and neutrinos and a variety of targets refined this picture and demonstrated small but systematic nonscaling behavior.The structure functions were shown to vary more rapidly with Q 2as x decreases,in accord with the nascent QCD prediction that the fundamental strong coupling constant αS varies with Q 2,and that at short distance scales (high Q 2)the number of observable partons increases due to increasingly resolved quantum fluc-tuations.Figure 1shows sample modern results for the Q 2dependence of the dominant structure function,in excellent accord with QCD predictions.The structure function values at all x depend on the quark content;the increases at larger Q 2depend on both quark and gluon content.The data permit the mapping of the proton’s quark and gluon content exemplified in Fig.2.2.Quark and gluon jetsThe gluon was firmly predicted as the carrier of the color force.Though its presence had been inferred because only about half the proton momentum was found in charged constituents,direct observation of the gluon was essential.This came from experiments at the DESY e +e −collider (PETRA)in 1979.The collision forms an intermediate virtual photon state,which may subsequently decay into a pair of leptons or pair of quarks.The colored quarks cannot emerge intact from the collision region;instead they create many quark-antiquark pairs from the vacuum that arrange themselves into a set of colorless hadrons moving approximately in the directions of the original quarks.These sprays of roughly collinear particles,called jets,reflect the directions of the progenitor quarks.However,the quarks may radiate quanta of QCD (a gluon)prior to formation of the jets,just as electrons radiate photons.If at sufficiently large angle to be distinguished,the gluon radiation evolves into a separate jet.Evidence was found in the event energy-flow patterns for the ‘three-pronged’jet topologies expected for events containing a gluon.Experiments at higher energy e +e −colliders illustrate this gluon radiation even better,as shown in Fig.3.Studies in e +e −and hadron collisions have verified the expected QCD structure of the quark-gluon couplings,and their interference patterns.FIG.3.A three jet event from the OPAL experiment at LEP.The curving tracks from the three jets may be associated with the energy deposits in the surrounding calorimeter,shown here as histograms on the middle two circles,whose bin heights are proportional to energy.Jets1and2contain muons as indicated,suggesting that these are both quark jets(likely from b quarks).The lowest energy jet3is attributed to a radiated gluon.3.Strong coupling constantThe fundamental characteristic of QCD is asymptotic freedom,dictating that the coupling constant for color interactions decreases logarithmically as Q2increases.The couplingαS can be measured in a variety of strong interaction reactions at different Q2scales.At low Q2,processes like DIS,tau decays to hadrons,and the annihilation rate for e+e−into multi-hadronfinal states give accurate determinations ofαS.The decays of theΥinto three jets primarily involve gluons,and the rate for this decay givesαS(M2Υ).At higher Q2,studies of the W and Z bosons(for example,the decay width of the Z,or the fraction of W bosons associated with jets)measureαS at the100GeV scale. These and many other determinations have now solidified the experimental evidence thatαS does indeed‘run’with Q2as expected in QCD.Predictions forαS(Q2),relative to its value at some reference scale,can be made within perturbative QCD.The current information from many sources are compared with calculated values in Fig.4.4.Strong interaction scattering of partonsAt sufficiently large Q2whereαS is small,the QCD perturbation series converges sufficiently rapidly to permit accurate predictions.An important process probing the highest accessible Q2 scales is the scattering of two constituent partons(quarks or gluons)within colliding protons and antiprotons.Figure5shows the impressive data for the inclusive production of jets due to scattered partons in pp collisions reveals the structure of the scattering matrix element.These amplitudes are dominated by the exchange of the spin1gluon.If this scattering were identical to Rutherford scattering,the angular variable0.10.20.30.40.511010FIG.4.The dependence of the strong coupling constant,αS ,versus Q using data from DIS structure functions from e ,µ,and νbeam experiments as well as ep collider experiments,production rates of jets,heavy quark flavors,photons,and weak vector bosons in ep ,e +e −,and pt ,is sensitive not only to to perturbative processes,but reflectsadditional effects due to multiple gluon radiation from the scattering quarks.Within the limited statistics of current data samples,the top quark production cross section is also in good agreement with QCD.FIG.6.The dijet angular distribution from the DØexperiment plotted as a function ofχ(see text)for which Rutherford scattering would give dσ/dχ=constant.The predictions of NLO QCD(at scaleµ=E T/2)are shown by the curves.Λis the compositeness scale for quark/gluon substructure,withΛ=∞for no compositness(solid curve);the data rule out values of Λ<2TeV.5.Nonperturbative QCDMany physicists believe that QCD is a theory‘solved in principle’.The basic validity of QCD at large Q2where the coupling is small has been verified in many experimental studies,but the large coupling at low Q2makes calculation exceedingly difficult.This low Q2region of QCD is relevant to the wealth of experimental data on the static properties of nucleons,most hadronic interactions, hadronic weak decays,nucleon and nucleus structure,proton and neutron spin structure,and systems of hadronic matter with very high temperature and energy densities.The ability of theory to predict such phenomena has yet to match the experimental progress.Several techniques for dealing with nonperturbative QCD have been developed.The most suc-cessful address processes in which some energy or mass in the problem is large.An example is the confrontation of data on the rates of mesons containing heavy quarks(c or b)decaying into lighter hadrons,where the heavy quark can be treated nonrelativistically and its contribution to the matrix element is taken from experiment.With this phenomenological input,the ratios of calculated par-tial decay rates agree well with experiment.Calculations based on evaluation at discrete space-time points on a lattice and extrapolated to zero spacing have also had some success.With computing advances and new calculational algorithms,the lattice calculations are now advanced to the stage of calculating hadronic masses,the strong coupling constant,and decay widths to within roughly10–20%of the experimental values.The quark and gluon content of protons are consequences of QCD,much as the wave functions of electrons in atoms are consequences of electromagnetism.Such calculations require nonperturbative techniques.Measurements of the small-x proton structure functions at the HERA ep collider show a much larger increase of parton density with decreasing x than were extrapolated from larger x measurements.It was also found that a large fraction(∼10%)of such events contained afinal。
宇宙中的黑暗物质:解读物质组成的谜题

宇宙中的黑暗物质:解读物质组成的谜题1. 引言1.1 概述:宇宙中的黑暗物质一直是天文学界的一个谜题。
尽管已经确定黑暗物质存在,并且占据着宇宙中绝大部分的物质组成,但我们对于其究竟是什么以及如何与其他物质相互作用的理解仍然有限。
本文将深入探讨黑暗物质的发现、性质以及当前研究进展和挑战。
1.2 文章结构:本文首先回顾了黑暗物质的发现过程和历史背景。
我们将看到黑暗物质究竟是如何被科学家们发现的,并了解相关研究在推动理论和观测方面的突破。
接下来,我们将详细讨论黑暗物质的性质和特征,包括不同科学观点下的理论模型以及目前采用的探测方法。
在第四节中,我们将介绍当前关于黑暗物质研究的最新进展和面临的挑战,从观测数据分析、实验验证困难以及理论探索方向等方面进行阐述。
最后,在结论与展望部分中,我们将总结研究成果,并展望未来对黑暗物质的深入研究,以及这一科学探索对于理解宇宙和揭示其真相的重要性。
1.3 目的:本文旨在通过对宇宙中的黑暗物质进行详细解读,帮助读者更好地理解人类在这个领域所取得的进展和挑战。
同时,我们也希望激发更多科学家参与到黑暗物质研究中来,共同推动对于这一谜团的探索。
通过该文章,读者将能够了解黑暗物质的基本概念、发现历史、性质特征以及当前研究所面临的问题和可能的突破方向。
这将为读者提供关于黑暗物质相关知识的全面介绍,并引导他们思考关于宇宙构成和演化过程等大问题。
2. Discovery and History of Dark Matter2.1 Discovery Process:The existence of dark matter was first suggested by the Swiss astronomer Fritz Zwicky in the 1930s. Zwicky observed the velocities of galaxies within the Coma Cluster and noticed that their speeds were much higher than what could be accounted for by visible matter alone. He proposed that there must be some invisible form of matter, which he called "dunkle Materie" or dark matter, exerting gravitational forces to explain this discrepancy.Further developments in the study of dark matter came in the late 20th century when astronomers began measuring the rotation curves of galaxies. Vera Rubin and Kent Ford found that stars on the outer edges of spiral galaxies were moving at unexpectedly high speeds, defying thepredictions based on visible mass distribution. Their observations provided additional evidence for the presence of dark matter.2.2 Historical Background:The concept of dark matter gained significant traction during the latter half of the 20th century. Astronomers started conducting various experiments and observations to understand its nature and composition better.One crucial milestone came with measurements taken by NASA's Cosmic Background Explorer (COBE) mission in 1990. The mission detected tiny fluctuations in temperature across cosmic microwave background radiation, which is considered one of the strongest pieces of evidence for both dark matter and its companion component, dark energy.2.3 Significance of Research:The discovery and research on dark matter are pivotal to our understanding of the universe's structure, formation, and evolution. Dark matter dominates over visible matter by approximately five times, implying that it plays a fundamental role in shaping galaxies' dynamicsand large-scale structures in space.Moreover, understanding dark matter can potentially provide insights into particle physics beyond what has been observed by colliders such as CERN's Large Hadron Collider (LHC). Unraveling its properties can shed light on new physics theories like supersymmetry and help answer questions related to the fundamental nature of matter.Undoubtedly, further exploration and investigations into dark matter will continue to shape our comprehension of the cosmos, ultimately leading to breakthroughs in astrophysics and particle physics.Note: The given content is a general overview of the "Discovery and History" section.3. 黑暗物质的性质与特征:3.1 科学观点:在当前的宇宙学理论中,黑暗物质被认为是构成宇宙大部分物质的一个关键组成部分。
带电粒子和球体电场,英语口语作文

带电粒子和球体电场,英语口语作文Title: The Interplay of Charged Particles and Spherical Electric Fields.In the vast and intricate landscape of physics, the interaction between charged particles and electric fieldsis a fascinating and fundamental aspect. This intricate dance between positive and negative charges, and the resulting electric fields they generate, is crucial in understanding the behavior of matter and energy. Specifically, when we delve into the realm of spherical electric fields, the complexity and beauty of these interactions become even more apparent.Let us delve into the world of charged particles. These particles, whether electrons, protons, or ions, possess an electric charge that determines their interaction with other charged particles and electric fields. The charge can be positive or negative, and its magnitude is measured in Coulombs. Charged particles are constantly in motion,influenced by the electric forces acting upon them.A spherical electric field, on the other hand, is created when a charge is distributed uniformly over the surface of a sphere. This distribution generates anelectric field that radiates outward from the center of the sphere, resembling the spokes of a wheel. The strength of this field decreases as one moves away from the center, following an inverse square law.When charged particles encounter such a spherical electric field, their trajectory is altered. If theparticle is positively charged, it will be attracted towards the negative charge distribution on the sphere. Conversely, a negatively charged particle will be repelled by the negative charge on the sphere. The force acting on the particle is proportional to the charge on both the particle and the sphere, as well as the inverse square of the distance between them.This interaction becomes particularly interesting when considering the dynamics of charged particles within thefield. For instance, a positively charged particle approaching a negatively charged sphere will accelerate towards it, reaching a maximum speed as it nears the surface. However, as it passes the sphere, the force acting on it reverses direction, causing it to decelerate and then accelerate back towards the original direction. This oscillatory motion continues indefinitely, with theparticle oscillating back and forth between the sphere and infinity.On the other hand, a negatively charged particle approaching a negatively charged sphere will be repelled and move away from the sphere. Its speed will increase asit moves away, but eventually, the force acting on it will become too weak, and the particle will coast along a straight path.The implications of these interactions are profound. They play a crucial role in understanding the behavior of atoms, molecules, and even larger objects like planets and stars. For instance, the electrostatic forces between charged particles are responsible for the stability ofatomic structures and the bonds that hold molecules together. Similarly, the electric fields generated by charged bodies like planets and stars influence the motion of other objects in their vicinity.In conclusion, the interplay between charged particles and spherical electric fields is a fascinating and crucial aspect of physics. It underpins our understanding of the behavior of matter and energy at the fundamental level. As we continue to explore this intricate dance between charges and fields, we gain deeper insights into the secrets of the universe and the nature of reality itself.。
有关科学的发现的英语作文

有关科学的发现的英语作文The Discovery of Science。
Science is a constantly evolving field, with new discoveries and breakthroughs happening all the time. These discoveries have the potential to change the way we understand the world and improve our lives in countless ways. In this essay, we will explore some of the most significant discoveries in the field of science and their impact on society.One of the most important discoveries in recent yearsis the detection of gravitational waves. Predicted byAlbert Einstein over a century ago, these ripples in the fabric of spacetime were finally observed in 2015 by the Laser Interferometer Gravitational-Wave Observatory (LIGO). This discovery has opened up a whole new way of observing the universe, allowing scientists to study phenomena such as black holes and neutron stars in ways that were previously impossible. It has also confirmed a keyprediction of Einstein's theory of general relativity, further validating our understanding of the fundamental laws of the universe.Another groundbreaking discovery is the development of CRISPR-Cas9 gene editing technology. This revolutionarytool allows scientists to precisely edit the DNA of living organisms, opening up new possibilities for treating genetic diseases and creating genetically modified organisms. The potential applications of this technology are vast, from curing genetic disorders to creating crops that are more resistant to disease and climate change. However, it also raises ethical questions about the potential misuse of this powerful tool and the need for careful regulation and oversight.In the field of medicine, the discovery of the CRISPR-Cas9 gene editing technology has also led to the development of new cancer treatments. Immunotherapy, which harnesses the body's own immune system to fight cancer, has shown great promise in treating a variety of cancers. This approach has the potential to revolutionize cancertreatment, offering new hope to patients who previously had few options. In addition, advances in personalized medicine, such as the use of genetic testing to tailor treatments to individual patients, are leading to more effective and targeted therapies.In the realm of space exploration, the discovery of exoplanets – planets orbiting stars outside our solar system – has captured the public's imagination. The Kepler space telescope has identified thousands of exoplanets, some of which may have the potential to support life. This has sparked renewed interest in the search for extraterrestrial life and has raised important questions about our place in the universe.The discovery of the Higgs boson, often referred to as the "God particle," is another major milestone in the field of particle physics. This elusive particle, which was predicted by the Standard Model of particle physics, was finally observed in 2012 at the Large Hadron Collider. Its discovery has confirmed our understanding of the fundamental forces and particles that make up the universe,and has opened up new avenues for exploring the nature of matter and energy.These are just a few examples of the many groundbreaking discoveries that have shaped our understanding of the world and have the potential to transform our lives in the future. As science continues to advance, we can expect even more exciting and impactful discoveries to come. These discoveries will not only expand our knowledge of the universe, but also have the potentialto improve our health, our environment, and our way of life. It is essential that we continue to support and invest in scientific research, as the benefits of these discoveries are boundless.。
未来的物理英文作文

未来的物理英文作文Title: The Future of Physics: Exploring Frontiers Beyond the Horizon。
In the ever-evolving landscape of human knowledge, physics stands as a beacon of enlightenment, constantly pushing the boundaries of what we understand about the universe. As we gaze into the future, the trajectory of physics appears both promising and enigmatic, promising new revelations while presenting daunting challenges. This essay endeavors to explore the potential avenues of inquiry and discovery that await us in the realm of physics.One of the most tantalizing frontiers in physics liesin the realm of quantum mechanics. Since its inception, quantum theory has revolutionized our understanding of the microscopic world, challenging conventional notions of causality and determinism. Looking forward, the quest to reconcile quantum mechanics with the theory of general relativity remains one of the most pressing issues intheoretical physics. The elusive theory of quantum gravity, which seeks to unite these two pillars of modern physics, holds the key to unlocking the mysteries of black holes, the early universe, and the nature of space-time itself.Furthermore, the advent of quantum computing heralds a new era of computational power, promising to revolutionize fields ranging from cryptography to drug discovery. Quantum computers, harnessing the strange phenomena of superposition and entanglement, possess the potential to solve problems that are currently intractable for classical computers. As researchers continue to refine quantum algorithms and hardware, we stand on the brink of a technological revolution that will redefine the limits of human ingenuity.In parallel, the exploration of the cosmos remains a central endeavor of modern physics. From the enigmatic dark matter and dark energy that dominate the universe's composition to the search for habitable exoplanets beyond our solar system, astronomers and cosmologists are probing the furthest reaches of space and time. The next generationof telescopes, such as the James Webb Space Telescope and the Square Kilometre Array, promise to unveil new vistas of the cosmos, shedding light on the origins and ultimate fate of the universe.Moreover, the intersection of physics with other disciplines, such as biology and computer science, continues to yield fruitful insights. The emerging field of biophysics seeks to understand the fundamental physical principles underlying biological processes, from thefolding of proteins to the dynamics of neural networks. Similarly, the field of quantum biology explores how quantum phenomena, such as coherence and tunneling, may play a role in biological systems, offering new perspectives on the nature of life itself.However, amidst the excitement of exploration, physicists also face ethical and existential questions. The development of powerful technologies, such as artificial intelligence and gene editing, raises concerns about the unintended consequences and ethical implications of our scientific pursuits. As we venture into the unknown, it isimperative that we proceed with caution, mindful of the potential risks and societal implications of our discoveries.In conclusion, the future of physics holds boundless possibilities, from unraveling the mysteries of the quantum world to exploring the farthest reaches of the cosmos. As we navigate this uncharted territory, we must remain guided by the principles of curiosity, humility, and ethical responsibility. For it is through our collective efforts and relentless pursuit of knowledge that we will continue to illuminate the darkest corners of the universe and unlock the secrets of existence itself.。
The mysteries of the atom Quantum mechanics

The mysteries of the atom QuantummechanicsQuantum mechanics, the branch of physics that deals with the behavior of particles at the atomic and subatomic level, has long been a subject offascination and mystery. From the early days of its development in the early 20th century, it has challenged our understanding of the fundamental nature of reality and introduced concepts that are often counterintuitive and difficult to grasp. One of the key mysteries of quantum mechanics is the wave-particle duality. Unlike classical physics, where particles are seen as distinct entities with definite positions and velocities, quantum mechanics tells us that particles can also behave as waves. This means that they can exhibit interference patterns, similarto the ripples in a pond when two waves meet. This duality is exemplified by the famous double-slit experiment, where particles such as electrons or photons are sent through two slits and create an interference pattern on a screen, as if they were waves. This baffling behavior challenges our conventional understanding of particles and forces us to rethink the nature of reality. Another enigma of quantum mechanics is the concept of superposition. According to quantum theory, particles can exist in multiple states simultaneously. This means that an electron, for example, can be in a state of both spin up and spin down at the same time. Itis only when we measure the particle that it "chooses" one of the possible states. This idea of superposition is at the heart of quantum computing, where quantum bits, or qubits, can exist in a superposition of both 0 and 1, allowing for exponentially faster calculations. However, the exact mechanism behind superposition and the collapse of the wave function upon measurement is still not fully understood. Quantum entanglement is yet another puzzling phenomenon in the realm of quantum mechanics. It refers to a situation where two or more particles become linked in such a way that the state of one particle is instantly correlated with the state of the other, regardless of the distance between them. This instantaneous connection, often referred to as "spooky action at a distance" by Albert Einstein, challenges our understanding of cause and effect and raises questions about the nature of reality itself. Despite numerous experimentsconfirming the existence of entanglement, its underlying mechanism remains elusive. The mysteries of quantum mechanics have not only captivated scientists but also sparked philosophical debates about the nature of reality. The Copenhagen interpretation, proposed by Niels Bohr and his colleagues, suggests that particles do not have definite properties until they are measured. This view emphasizes the role of the observer in determining the outcome of an experiment and raisesprofound questions about the nature of consciousness and the limits of human knowledge. On the other hand, the Many-Worlds interpretation, put forward by Hugh Everett, suggests that every possible outcome of a quantum event actually happensin a separate universe, leading to a multiverse of parallel realities. The mysteries of the atom and quantum mechanics continue to perplex and intrigue scientists and philosophers alike. While significant progress has been made in understanding the behavior of particles at the quantum level, there are still many unanswered questions. The quest to unravel these mysteries has led to the development of new technologies such as quantum computing and quantum cryptography, which have the potential to revolutionize fields such as information processingand secure communication. As we delve deeper into the quantum realm, we mayuncover even more astonishing phenomena and gain a deeper understanding of the fundamental building blocks of our universe.。
Particle and Nuclear Physics
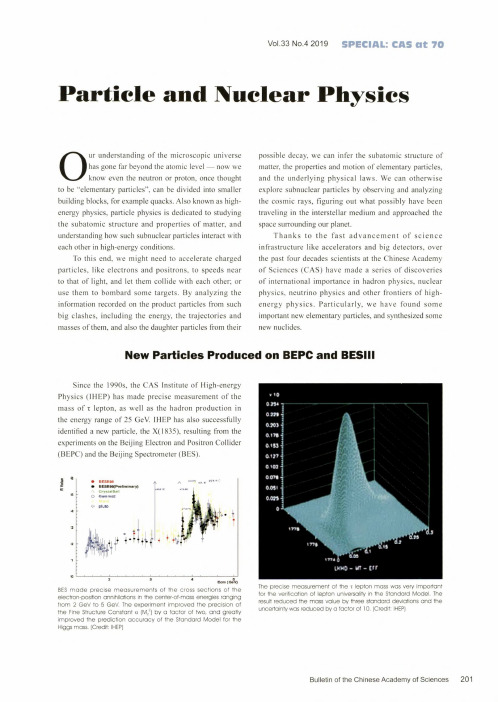
Particle and Nuclear PhysicsOur understanding of the microscopic universe has gone far beyond the atomic level — now weknow even the neutron or proton, once thoughtto be "elementary particles", can be divided into smaller building blocks, for example quacks. Also known as high- energy physics, particle physics is dedicated to studying the subatomic structure and properties of matter, andunderstand! n g how such sub n uclear particles in t eract with each other in high-energy conditions.To this end, we might need to accelerate charged particles, like electrons and positrons, to speeds near to that of light, and let them collide with each other; oruse them to bombard some targets. By analyzing theinformation recorded on the product particles from suchbig clashes, including the energy, the trajectories and masses of them, and also the daughter particles from theirpossible decay, we can infer the subatomic structure ofmatter, the properties and motion of elementary particles, and the underlying physical laws. We can otherwiseexploresubnuclear particles by observing and analyzing the cosmic rays, figuring out what possibly have beentraveling in the interstellar medium and approached the space surrounding our planet.Thanks to the fast advancement of science infrastructure like accelerators and big detectors, overthe past four decades scientists at the Chinese Academyof Sciences (CAS) have made a series of discoveries of in t ernational importance in hadron physics, nuclear physics, neutrino physics and other frontiers of high- energy physics. Particularly, we have found someimportant new elementary particles, and synthesized some new nuelides.New Particles Produced on BEPC and BESIIISince the 1990s, the CAS Institute of High-energyPhysics (IHEP) has made precise measurement of the mass of lepton, as well as the hadron production inthe energy range of 25 GeV. IHEP has also successfully identified a new particle, the X(1835), resulting from theexperiments on the Beijing Electron and Positron Collider (BEPC) and the Beijing Spectrometer (BES).BES made precise measurements of the cross sections of the electron-position annihilations in the center-of-mass energies ranging from 2 GeV to 5 GeV. The experiment improved the precision of the Fine Structure Constant a (M z 2) by a factor of two, and greatly improved the prediction accuracy of the Standard Model for the Higgs mass. (Credit: IHEP)* i 1The precise measurement of the t lepton mass was very important for the verification of lepton universality in the Standard Model. The result reduced the mass value by three Standard deviations and the uncertainty was reduced by a factor of 10. (Credit: IHEP)0 3.7 3.8 3.9 4.0Mmax仗士J")(GeV/c2)An illustration of Z c(3900),the tetraquack particle identified on BESIII(Credit:IHEP)The tetraquack hadron4(3900)discovered on BESIII(Image:IHEP)In2013,an experiment joined by over350physicists from11countries on BESIII,the upgraded version of BESII,led to the first observation of a“tetraquack"hadron Z c(3900),subverting the traditional belief that a particle of matter could only be composed of either two or three quacks. This discovery was ranked first among the11important achievements picked out by Physics,a journal sponsored by the American Physical Society in the same yea匚Synthesis of New Super-heavy Isotopes at HIRFLIn1992,scientists from the CAS Shanghai Institute of Nuclear Research(SINR)obtained a new nuclide,the Platinum-202(202Pt)on the Heavy Ion Research Facility at Lanzhou(HIRFL),which produces medium-to high-energy ion beams.This success marked the first new isotope synthesized by Chinese scientists.Since the1990s,another institution under the umbrella of CAS,the Institute of Modern Physics(IMP) in Lanzhou,Gansu Province has artificially synthesized a total of34new nuclides,and successfully measured the masses of a series of short-lived nuclei with high precision.IMP has also established an international evaluation center for data on nuclei masses.Anti-helium-4,the Heaviest Anti-nucleusIn2011,the international community of particle physicists scored a milestone achievement-the successful identification of the heaviest anti-matter particle,the anti-helium-4(anti-4H).The international team working at the STAR detector of RHIC,the Relativistic Heavy Ion Collider at the U.S.Department of Energy's Brookhaven National Laboratory(BNL),identified this anti-nucleus and described the discovery in a paper published in Nature on April24,2011.As partners in the twelve-country STAR collaboration,several CAS Institutions,including the CAS Shanghai Institute of Applied Physics(SINAP),the heirs of SINR,the University of Scienee and Technology of China(USTC),and the Institute of Modern Physics100cmThe STAR collaboration detected18examples of the unique"signature" of the antihelium-4nucleus based on the Time of Flight(TOF)detector, which was designed and made by the STAR TOF project team from China and US.(Credit:SINAP)(IMP), played a key role in the discovery. The Chinese team designed and built the Time of Flight detector of RHIC, based on which the measurement of anti-4H, alsoknown as anti-alpha, was made. The Chinese team also attended the data processing of the experiment.RHIC is a particle accelerator used to simulate andstudy conditions of the early universe just after the Big Bang. The research is expected to shed some light on oneof the greatest mysteries of physics: Why our universe appears to be made entirely of ordinary matter, whilstmatter and antimatter are understood to have been createdin equal amounts at the time of the Big Bang.New “Flavor ” of NeutrinoThe year 2012 witnessed the discovery of a new "flavor ” of neutrino - the third type of neutrino oscillationas a result from the work at Daya Bay Neutrino Experimentheaded by IHEP.The Daya Bay Neutrino Experiment, based at the Daya Bay nuclear power plant in Guangdong Province ofsouthern China, started running in 2011. In March 2012, the experimental collaboration announced the discoveryof the third type of neutrino oscillation, and also the precise measurement of the neutrino mixing angle 0n .This discovery, listed among the annual 10 sciencebreakthroughs by Science in 2012, earned the team the Breakthrough Prize for Fundamental Physics in 2016.The team was also awarded the First Prize of the National S&T Awards for Natural Science.To further explore neutrino physics, a much biggerneutrino experiment, the Jiangmen Underground NeutrinoObservatory (JUNO) is currently under construction at a site near to the city of Jiangmen in Guangdong Province.The neutrino detector immersed in water at the Daya Bay Neutrino Experiment (Image: IHEP)A total of 192 eight-inch photomultipliers are installed on the inner wall of the neutrino detector of Daya Bay Neutrino Experiment (DBNE), to pick up the dim signals given off by the neutrinos caught by the detector.On March 8, 2012, the DBNE collaboration announeed the discovery of a new type of neutrino oscillation. The team's first results indicated that the neutrino mixing angle theta 13, expressed as sin 22G )3, is equal to 0.092 plus or minus 0.017. (Credit: IHEP)CAS's Role in Higgs DetectionThe intemational experiment and research occurringin2012on the Large Hadron Collider(LHC)of CERN(the European Organization for Nuclear Research)thatsuccessfully detected Higgs boson,the"last missing brick,^of the Standard Model,involved thousands of scientistsfrom over a hundred institutes in30countries,includingChinese institutions headed by IHEP and USTC.Chinese scientists directly contributed to the buildingof two LHC detectors:CMS(the Compact Muon Solenoid)and ATLAS(A Toroidal LHC Apparatus).The former isa general-purpose detector aimed at searching of Higgs,the"God Particle",and the latter targeted at new physicsbeyond the Standard Model.Chinese scientists alsoprovided a smart method to differentiate the target particle, which occurs only once out of a trillion collisions,from the massive number of hadrons and quantum photons produced by the mini artificial tfc Big Bang"in the big collider.Triumph of the Standard Model:In2012,data from CERN's CMS and ATLAS experimen恰strongly suggested that a new subatomic particle, which appeared to be in good consistence to the Iong-sought-after Higgs boson,was detected.(Image:CERN)。
Fundamental Forces and Particle Physics

Fundamental Forces and ParticlePhysicsThe universe around us is governed by four fundamental forces: gravity, electromagnetic, weak, and strong forces. These forces hold everything in our world together, from small subatomic particles to the largest celestial bodies in the universe. Understanding these forces is essential to comprehend the workings of the universe. Particle physics is the study of subatomic particles and their interactions with these fundamental forces.Gravity is the force that holds the universe together. It is the weakest of all fundamental forces, but it is crucial for understanding the structure of the universe. Gravity is responsible for holding planets, stars, and galaxies in place. The force is proportional to the masses of the two objects and depends on the distance between them. Gravity is unique because it acts on an object's mass, regardless of its charge.The electromagnetic force is responsible for the interaction between charged particles. It is the force that holds atoms together and controls interactions between molecules. Electromagnetic force is also responsible for electric and magnetic fields and the formation of light. The electromagnetic force is much stronger than gravity and can overcome the gravitational force between two objects. It can also cause the repulsion or attraction of charged particles, depending on their charges.The weak force is one of the four fundamental forces and is responsible for beta decay, one of the ways that atoms undergo radioactive decay. It is the force responsible for turning a neutron into a proton, an electron, and an antineutrino. The weak force can only act over short distances and is significantly weaker than both the electromagnetic and strong forces.The strong force is the strongest and shortest-ranged of the four fundamental forces. It is responsible for keeping protons and neutrons together in an atomic nucleus. The strong force is what holds the nucleus together and opposes the electric force of repulsionbetween protons. Without the strong force, atoms would not exist as we know them, and matter would not be able to form.Particle physics studies the particles that make up matter and the interactions between them. Subatomic particles include electrons, protons, and neutrons, as well as elementary particles like quarks, leptons, and bosons. Bosons are force-carrying particles that carry the fundamental forces. They include the photons that carry electromagnetic force and the W and Z bosons that carry the weak force.Particle physics has helped us understand the behavior of matter and energy at the smallest and most fundamental levels. The discoveries made in particle physics have led to many applications, such as particle accelerators and medical imaging technologies. Particle physics is an important field of study, and as we continue to explore new frontiers, it's an area that will never cease to amaze us.To conclude, fundamental forces and particle physics are essential to understanding the world around us. The universe is governed by four fundamental forces that hold everything in the world together. These forces have varying strengths and different distances over which they can act. Particle physics studies the subatomic particles that make up matter and the interactions between them. It has led to many new discoveries and applications. Understanding these forces and particles is essential to comprehend the workings of the universe and opens up new possibilities for exploration.。
粒子治疗设备英语作文

粒子治疗设备英语作文Particle therapy, a groundbreaking approach to cancer treatment, is revolutionizing the way we combat this deadly disease. This advanced medical technology utilizes subatomic particles, such as protons or carbon ions, to preciselytarget and destroy cancer cells while minimizing damage tothe surrounding healthy tissues. The allure of particletherapy lies in its unparalleled precision and the profound impact it has on improving patient outcomes.At the heart of this technology are particle accelerators, which generate high-energy beams capable of penetrating deep into the body to reach even the most elusive tumors. The unique physics of particle therapy allows for a phenomenon known as the Bragg peak, where the energy of the particle beam is concentrated at a specific depth, delivering a maximum dose of radiation to the tumor while sparing thetissue beyond it. This is a stark contrast to conventional radiation therapy, which distributes radiation along theentire path of the beam.The clinical benefits of particle therapy are manifold.It offers a less invasive alternative to surgery forinoperable tumors, reduces the risk of secondary cancers caused by radiation exposure, and is particularly effectivefor pediatric patients, where minimizing long-term effects is paramount. Moreover, for tumors located near critical structures such as the brain or spinal cord, particle therapystands out as a safer option.Despite its many advantages, particle therapy is not without its challenges. The high cost of equipment and the complexity of treatment planning can be prohibitive for many healthcare facilities. Additionally, the availability of this treatment is limited, with only a handful of centers worldwide equipped to offer it.As research and development in the field of particle therapy continue to advance, we stand on the cusp of a new era in cancer care. With ongoing clinical trials exploring the potential of this technology for an even broader range of cancers, the future holds promise for a more personalized and effective approach to treatment. The quest for a cure is relentless, and particle therapy is a beacon of hope in this ongoing battle against cancer.。
particle例句

particle例句1. “I was learning about particles in science class. The teacher was talking about how tiny they are. I was like, ‘Whoa, these particles are so small we can't even see them with our naked eyes!’It was really mind-boggling.”(我在科学课上学习关于粒子的知识。
老师在讲它们有多小。
我就想,“哇,这些粒子太小了,我们用肉眼甚至都看不见它们!”这真的让人难以置信。
)上科学课学粒子的时候,老师说它们可小了。
我就觉得,哎呀,这么小,肉眼都看不见。
Particle 这东西真神奇。
2. “My friend tried to explain particles to me in a simple way. He said, ‘Think of particles like little tiny dots that make up everything.’I nodded, but still, it was a bit hard to fully grasp. But it was fun trying to understand these mysterious particles.”(我朋友试图用简单的方式给我解释粒子。
他说,“把粒子想象成组成一切的小点点。
”我点点头,但还是有点难完全理解。
不过试着理解这些神秘的粒子还挺有趣的。
)朋友给我讲粒子,说就像小点点组成了所有东西。
我似懂非懂的,不过琢磨这些粒子还挺有意思。
Particle 有点神秘哦。
3. “I read a book about particles and it said that they behave in really strange ways. Like they can be in two places at once. That's just crazy! I couldn't help but wonder how something so small could have such strange properties. Particles are really something else.”(我读了一本关于粒子的书,它说它们的行为方式非常奇怪。
A-LEVEL A2 物理复习资料 particle physics-推荐下载

Section3 particle physics1.The nuclear atom(1)Mass number and atomic number(2)Alpha particle scattering experiment(3)Electrons are accelerated by E and B(4)Write equations using standard nuclear notating(5)Explain why high energies are required to break particles into their constituents and to investigate fine structure(6) de Broglie wavelength λ=h/p(201306R-7)The de Broglie wavelength associated with electrons moving at 2.5 × 106 m s–1 isA .2.9 × 10–4mB .2.4 × 10–8 mC .2.9 × 10–10mD .2.4 × 10–39m (201306-10) The de Broglie wavelength for neutrons used to study crystal structure is 1.2 nm. mass of a neutron = 1.67 × 10–27kgThe speed of these neutrons would beA .3.0 × 106 m s–1 B.3.3 × 102m s–1 C.3.0 × 10–3m s–1 D.3.3 × 10–7m s–1 (201306R-8 )Which of the following is not a valid conclusion from Rutherford’s alpha particle scattering experiment?A .The atom is mainly empty space.B .The mass of the atom is mostly concentrated in the nucleus.C. The nucleus must be positively charged.D .The nucleus must be very small compared to the atom.(201306R-9) Select the row in the table that correctly identifies the composition of a 235U+ ion.92Number of protons Number of neutrons Number of electrons A9214391B9214392C9223591D9323592(201306-1)The nucleus of one of the isotopes of nickel is represented by 6028Ni. Which line correctly identifies a neutral atom of this isotope?Number of protons Number of neutrons Number of electrons A283228B283232C286028D602828(201201-10)The de Broglie wavelength of a moving tennis ball is calculated as 1× 10-33 m. This means that the moving tennis ballA .diffracts through a narrow slit. B.does not behave as a particle.C.does not display wave properties.D.is travelling at the speed of light.(201306R-10) .A transmissionelectron microscope passes abeam of electrons through atiny specimen to form an imageon a viewing screenDue to the wave nature ofelectrons, diffraction occurswhich can blur the image. Toreduce this effect whenviewing a smaller object thebeam must containA .more electrons per second.B .fewer electrons per second.C. faster moving electrons. D .slower moving electrons(201306-13).In an experiment to investigate the structureof the atom, α-particles are fired at a thin metal foil,which causes theα-particles to scatter.(a)(i) State the direction in which thenumber of α-particles detected will be amaximum(ii) State what this suggests about the structure of the atoms in the metal foil.(b) Some α-particles are scattered through180°.State what this suggests about the structure of the atoms in the metal foil.(c)The diagram shows the path of anα-particle passing near to a single nucleus inthe metal foil.(i)Name the force that causesthe deflection of the α-particle.(ii) On the diagram, draw anarrow to show the direction of the force acting on the α-particle at the point where the force is a maximum. Label the force F.(iii) The foil is replaced by a metal of greater proton number.Draw the path of an α-particle that has the same initial starting point and velocity as the one drawn in the diagram.(201301-11) Early in the twentieth century physicists observed the scattering of alpha particles after they had passed through a thin gold foil. This scattering experiment provided evidence for the structure of the atom.(a) State why it is necessary to remove the air from the apparatus that is used for this experiment.(b) From the results of such an experiment give two conclusions that can be deduced about the nucleus of an atom.Conclusion 1Conclusion 2(c) The diagram shows three α-particles ,allwith the same kinetic energy .The path followedby one of the particles is shown . Add to thediagram to show the paths followed by the othertwo particles.(201302-12 )The electron in a hydrogen atom can be described by a stationary wave which is confined within the atom.This means that the de Broglie wavelengthassociated with it must be similar to the size of the atom which is of the order of 10–10 m.(a)(i)Calculate the speed of an electron whose de Broglie wavelength is1.00 × 10–10m. (ii) Calculate the kinetic energy of this electron in electronvolts(b)When βradiation was first discovered ,it was suggested that there were electrons in the atomic nucleus, but it was soon realised that this was impossible because the energy of such an electron would be too great.Suggest why an electron confined within a nucleus would have a much greater energy than the energy calculated in (a)(ii).2.high-energy collisions(1)The role of E in linear particle accelerators(2)r=p/BQ in B(3)⊿E=c2⊿m(4)Units:MeV,GeV,MeV/c2 ,GeV/c2,u(201301-10)The tubes of a linear accelerator (linac) get progressively longer down its length becauseA .the accelerating particles become relativistic.B .the frequency of the applied potential difference changes.C .the accelerating particles must spend the same time in each tube.D .the accelerating particles gain mass(201206-10)As a particle accelerates in a linac, it passes through drift tubes of increasing lengths. This is so thatA.the particle can be given more energy within each tube.B.the frequency of the accelerating voltage can be constant.C.the accelerating voltage can be as high as possible.D.the time spent in the tube by the particle is longer(201306R-6)Charged particles are travelling at a speed v, at right angles to a magnetic field of flux density B. Each particle has a mass m and a charge Q.Which of the following changes would cause a decrease in the radius of the circular path of the particles?A .an increase inB B .an increase in mC .an increase in vD .a decrease in Q (201306-6)A muon has a mass of 106 MeV/c2. The mass of a muon, to two significant figures, isA .1.7 × 10–11 kgB .5.7 × 10–20 kgC .1.9 × 10–28 kg D.1.9 × 10–34 kg (201201-7)The rest mass of a proton is 1.67 × 10–27 kg. This mass, in MeV/c2 is approximatelyA .2.4 × 10–20 B.3.1 × 10–6 C.1.0 D .940(201106-10)The Large Hadron Collider is designed to accelerate protons to very high energies for particle physics experiments. Very high energies are required toA .annihilate hadrons. B.collide hadrons.C .create particles with large mass. D.produce individual quarks(201206-13) An electron and a positron annihilate with the emission of two photons of equal energy. Calculate the wavelength of the photons.(201201-17) (d) The technology suggested in the science fiction series, Star Trek, for powering the Starship Enterprise relied on antimatter. When an anti-hydrogen atom meets a hydrogen atom, they annihilate and produce energy.(i)How much energy, in joules, would be produced by the annihilation ofjust 1 milligram of anti-hydrogen atoms?(ii) Anti-protons are required to produce anti-hydrogen atoms. The total production of anti-protons on Earth over the past 25 years adds up to only a few nanograms.Suggest why so little anti-matter has been created3. Particle accelerators(1) E and B in cyclotron particle accelerators(2) Relativistic effects at speeds near c(201306-9)A cyclotron is a type of particle accelerator. It consists of two metal Dees which are connected to a high frequency voltage supply and are in a strong magnetic field. The particles change their speed becauseA.of the magnetic field they are in.B.the voltage supply is alternating.C.there is a potential difference between the two Dees.D.the magnetic field is at right angles to the Dees.(201306R-11)*11 The diagram shows the basic structure of a cyclotron.With reference to the magnetic field and the alternating potential difference explain how the cyclotron produces a beam of high speed particles.4. Particle theory(1) E and B in particle detectors(2) Charge energy and momentum are conserved in interactions between particles andhence interpret records of particle tracks(3) In the standard quark-lepton model each particle has a corresponding anti-particle meson:quark-anti-quark pairs baryon :quark triplets(4) Write and interpret equations using Standard symbols(201206-9)A pion can decay to produce two leptons. Which one of the following is possible?A.π+→e+ + v e B .π0→e– + v e C.π+→e++ e– D π0→π++ e–(201201-8)A positive kaon (K+) is a meson which includes a strange quark. Its structure could beA . u B. us C. D .usds s d d(201201-9) The K+ is likely to decay tov vA.π+ + μ– + μB. π++π0Cπ++π– D π0 + μ– +μ(201306-7 )The diagram shows the tracks from an event at a point P in a bubble chamber. A magnetic field is directed into the page. The tracks cannot show the production of a proton-antiproton pair with equal kinetic energies becauseA .the curvature is perpendicular to the magnetic field.B .the tracks curve in different directions.C. the tracks have different curvatures.D .there is no track before point P(201306-11)Scientists studying anti-matter recently observed the creation of a nucleus of anti-helium 4, which consists of two anti-protons and two anti-neutrons. The diagram represents the path of a proton through a magnetic field starting at pointX.Add to the diagram the path of an anti-helium 4 nucleus also startingat point X and initially travelling at the same velocity as theproton.Explain any differences between the paths.(201306-14)The photograph shows tracks in aparticle detector.(a) Explain the role of a magnetic field in a particledetector(b) Explain how you can tell that track XY was produced by a particle moving fromX to Y rather than from Y to X.(c)The particle that produced track XY was a π+.Deduce the direction of the magnetic field in the photograph(d) At Y, the π+ decayed into a positively charged muon (μ+) and a muon neutrino. The μ+ has a very short range before decaying into various particles, including a positron which produced the final spiral.(i) Give two reasons why you can deduce that the muon neutrino is neutral.(ii) Explain the evidence from the photograph for the production of the muon neutrino at Y.(201306R-14) .Hadrons are a group of particles composed of quarks. Hadrons can be either baryons or mesons.(a) (i) State the quark structure of a baryon.(ii) State the quark structure of a meson.(b) State one similarity and one difference between a particle and its antiparticle. Similarity:Difference :(c) (i) The table gives some of the properties of up, down and strange quarks.Type of quark Charge/e Strangenessu+2/30d-1/30s-1/3-1One or more of these quarks combine to form aΚ+, a meson with a strangeness of +1.Write down the quark combination of theΚ+..(ii)TheΚ+can decay in the following way Κ+→ µ++ vµK–is the antiparticle of the K+ . Complete the equation below by changing each particle to its corresponding antiparticle in order to show an allowed decay for the K–meson.Κ-→(iii)The rest mass of theΚ+ is 494 MeV/c2Calculate, in joules, how much energy is released if a Κ+ meets and annihilates a K–(201306-12)The table gives some of the properties of the up, down and strange quarks.Type of quark Charge/e Strangenessu+2/30d-1/30s-1/3-1There are nine possible ways of combining u, d and s quarks and their antiquarks to make nine different mesons. These are listed belowu d s d u s s u du u u d d d s s s (a) From the list select the four strange mesons and state the charge and strangeness of each of them.Meson Charge/e Strangeness(b) Some of the mesons in the list have zero charge and zero strangeness.Suggest what might distinguish these mesons from each other.*14 A bubble chamber is a particle detector which makes use of electric and magnetic fields. Explain the role of electric and magnetic fields in a particle detector. (201301-17)In 2011 physicists at the Relativistic Heavy Ion Collider (RHIC) announced the creation of nuclei of anti-helium-4 which consists of anti-protons and anti-neutrons instead of protons and neutrons(a) ‘Ordinary’ helium-4 is written as 42He.What do the numbers 4 and 2 represent?(b) In the RHIC experiment, nuclei of gold 19779Au travelling at speeds greater than2.99 × 108m s–1, in opposite directions, collided, releasing energies of up to 200 GeV. After billions of collisions, 18 anti-helium nuclei had been detected.(i) What is meant by ‘relativistic’ in the collider’s name?(ii) State why it is necessary to use very high energies in experiments such as these. (iii) Show that the mass of a stationary anti-helium nucleus is about 4 GeV/c2(iv) State why the small number of anti-helium nuclei produced only survive for a fraction of a second.(v) A slow moving anti-helium nucleus meets a slow moving helium nucleus. If they were to combine to produce 2 high energy gamma rays, calculate the frequency of each gamma ray.(c) There are two families of hadrons, called baryons and mesons. Baryons such asprotons are made of three quarks.(i) Describe the structure of a meson(ii) Up quarks have a charge of +2/3e and down quarks a charge of –1/3e.Describe the quark composition of anti-protons and anti-neutrons and use this to deduce the charge on each of these particles.(201206-18)18 (a) Physicists were able to confidently predict the existence of a sixth quark. State why(b) The mass of the top quark was determined by an experiment. Collisions between protons and anti-protons occasionally produce two top quarks.(i) How do the properties of a proton and an anti-proton compare?(ii) After the collision the two top quarks move in opposite directions with the same speed. Explain why.(c) The two top quarks decay rapidly into two muons and four jets of particles. These can be detected and their momenta measuredThe diagram shows an end-on view of the directions of the four jets (J1 to J4) of particles. The two muons are shown as μ1and μ2. A muon neutrino is also produced but cannot be detected, so is not shown. Each momentum is measured inGeV/c.(i)Explain why GeV/c is a valid unit for momentum.(ii) The vector diagram shown below is not complete. Add to the diagram arrows to represent the momenta of J3 and J4.(iii) Complete the diagram to determine the magnitude of the momentum of the muon neutrino.(iv) Show that the total energy of all the products of this event is about 300 GeV. (v) Deduce the mass of a top quark in GeV/c2.(vi) Suggest why it took a long time to find experimental evidence for the top quark.。
Details determine success or failure

Frontier Research and Applications in PhysicsPhysics is a fundamental science that seeks to understand thebehavior of matter and energy at their most basic level. At the forefront of physics research, scientists are exploring newfrontiers and pushing the boundaries of human knowledge. These advances have not only expanded our understanding of the universebut also led to groundbreaking applications that have transformed technology and society.One area of frontier research in physics is quantum mechanics, which deals with the behavior of matter and energy at the atomic and subatomic levels. Scientists are delving into the strange and counterintuitive phenomena of quantum mechanics, such asentanglement and superposition, to develop quantum computing and communication technologies with unprecedented capabilities. These advancements have the potential to revolutionize fields such as cryptography, data processing, and simulation.Another frontier in physics research is the exploration of the fundamental forces and particles that make up the universe. Thestudy of particle physics, including the search for new particlesand the understanding of their interactions, is conducted at large-scale facilities such as the Large Hadron Collider. These effortsaim to unravel the mysteries of dark matter and dark energy, which constitute the majority of the universe's mass and energy but remain elusive to direct observation.Astrophysics is yet another frontier of physics research, focusingon the study of celestial bodies and the universe as a whole. Advancements in observational techniques and instrumentation have allowed scientists to make groundbreaking discoveries, such as the detection of gravitational waves and the first image of a black hole. These developments have provided unprecedented insights into the nature of space, time, and the evolution of the cosmos.In addition to advancing our understanding of the natural world, frontier research in physics has led to numerous practical applications. For example, the development of new materials with unique properties, such as superconductors and metamaterials, has revolutionized fields like electronics, telecommunications, and medical imaging. Furthermore, advances in laser technology have enabled applications in fields as diverse as manufacturing, medicine, and defense.In conclusion, frontier research in physics continues to drivescientific and technological progress, expanding our understandingof the universe and leading to transformative applications. Thepursuit of new knowledge in physics not only enriches ourunderstanding of the natural world but also has the potential to revolutionize technology and society in ways that were once unimaginable.。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
a r X i v :h e p -e x /0210052v 1 22 O c t 2002PARTICLE PHYSICS AT FUTURE COLLIDERSJohn Ellis,Theoretical Physics Division,CERN,Geneva,SwitzerlandAbstractThe search for physics beyond the Standard Model moti-vates new high-energy accelerators,which will require high luminosities in order to produce interesting new heavy ing the Higgs boson and supersymmetry as ex-amples,we discuss the capabilities of the LHC and e +e −linear colliders in the TeV and multi-TeV energy ranges to discover and study new particles.CERN-TH/2002-292hep-ex/0210052Talk presented at the 26th Advanced ICFA Workshop on Nanometre-Size Colliding Beams,Lausanne,September20021THE NEED FOR NANOBEAMSThe primary motivation for future colliders,and the only one likely to find favour with funding agencies,is to search for new physics beyond the Standard Model.This may be done either by going to higher energies,or by collid-ing beams with higher luminosities in the energy range al-ready probed by previous colliders.In general,the cross sections for interesting new physics processes decrease at higher energies:σinteresting ∼1M 2new.(2)A suitable standard of comparison for high-energy futurecolliders is provided by LEP,which reached a maximum luminosity L ∼1032cm −2s −1at E CM ∼200GeV .The pair-production of heavy particles such as the W ±and Z 0at LEP 2was not overly generous,so we assume that a new collider should provide a similar number of events.In thiscase,its luminosity should increase as E 2CM compared to LEP.Thus,the LHC with a luminosity L ∼1034cm −2s −1will be able to produce pairs of new particles each weigh-ing ∼1TeV .Likewise,a linear e +e −collider operating at E CM ∼5TeV ,such as CLIC,should be designed with a luminosity L ∼1035cm −2s −1.Such large luminosities are also required for advanced ‘factories’at lower energies:a rule of thumb is that an n ’th-generation factory shouldhave a luminosity ∼10n times greater than the first col-lider to explore the same energy range.This is why the present B factories aim at L ∼1034cm −2s −1,and one talks about L ∼1035cm −2s −1for the next generation,et seq....Nanobeams will certainly be in great demand.2THE STANDARD MODEL OFPARTICLE PHYSICSLEP and the lower-energy colliders that preceded it have established beyond question the Standard Model of parti-cle physics.It comprises three generations of fundamen-tal fermions to make up the matter in the Universe,each consisting of two quarks,a neutrino and an electron-like charged lepton.Four fundamental forces act on these mat-ter particles:the electromagnetic,strong,weak and gravi-tational forces.Each of these is carried by messenger par-ticles:the photon,the gluons,the W ±and Z 0,and (we believe)the graviton,respectively.As seen in Fig.1,the experimental data from LEP agree (too)perfectly with the theoretical curves,at all energies up to above 200GeV [1].This sounds great,but there are plenty of questions left open by the Standard Model.1010101010Centre-of-mass energy (GeV)C r o s s -s e c t i o n (p b )Figure 1:Data from LEP and other e +e −experiments agree perfectly with the predictions of the Standard Model [1].Why are some fundamental particles,such as the photon and gluons,massless,while others are massive,weighing as much as good-sized nuclei in the cases of the W ±,Z 0and top quark?Are the different fundamental forces uni-fied,as long hoped by Einstein?Why are there so many different types of ‘elementary’particles?Could they all be composite states made out of more fundamental con-stituents?How to explain all the different parameters of the Standard Model:6quark masses,3charged-lepton masses,2weak-boson masses,4weak mixing angles and phases,3interaction strengths and a non-perturbativestrong-interaction vacuum parameter?These total 19pa-rameters,even without describing neutrino masses and mixing angles.Some more fundamental physics must surely lie beyond the Standard Model,and the next sections of this paper de-scribe some candidates for this new physics.3THE PROBLEM OF MASSThis is probably the most pressing problem raised by theStandard Model.Indeed,it can only be solved by introduc-ing new physics at some energy scale below ∼1TeV .The most likely culprit for generating particle masses is thought to be a Higgs boson with a mass in this range.A mass-less vector particle such as the photon has two polarization states:λ=−1,+1.On the other hand,a massive vector particle such as the W ±or Z 0must have three polarization states:λ=−1,0,+1.Thus,in order for a massless vector particle to acquire a mass,it must combine with some zero-polarization state,such as could beprovided by a spin-0field via the Higgs-Brout-Englert mechanism [2].In the Standard Model,the minimal such model contains a complex doublet of Higgs fields,with a total of four de-grees of freedom.Of these,three are eaten by the W ±and Z 0to become their third polarization states,leaving one degree of freedom to appear as a separate physical state,the Higgs boson.In order for it to perform its task of giv-ing masses to other particles,its couplings to them should be proportional to their masses:g H ¯f f ∝m f .However,the mass of the physical Higgs boson itself is not fixed in the Standard model without any extra input.Direct searches for the Higgs boson at LEP have established that the Higgs boson weighs more than 114.4GeV [3].Precision electroweak data from LEP and elsewhere also provide indirect information on the possi-ble mass of the Higgs boson,as seen in Fig.2[1].Quan-tum corrections in the Standard Model would disagree with the precision measurements unless the Higgs boson weighs less than 193GeV at the 95%confidence level,with a mass ∼115GeV being the most likely value,as seen in Fig.3[4].This probability distribution makes no use of the ‘hint’from direct Higgs searches at LEP of a signal at ∼116GeV [3].Now that LEP operations have been terminated,what are the prospects for Higgs searches with future colliders?The Tevatron collider has a chance,if it can accumulate suf-ficient luminosity,particularly if the Higgs boson weighs ∼115GeV [5].The LHC will be able to discover the Higgs boson,whatever it mass below about 1TeV ,as well as observe two or three of its decay modes and measure its mass to 1%or better,as seen in Fig.4[6].The days of the Higgs boson are numbered!4SUPERSYMMETRYThe Higgs boson is confidently expected even within the Standard Model,but theorists think it should also bem H [GeV ]Figure 2:The χ2curve for a global fit to the precision elec-troweak data from LEP and elsewhere,with the uncertain-ties shaded in blue [1],favour a relatively light Higgs bo-son with mass close to the range excluded by experiment,shaded in yellow [3].Figure 3:An estimated probability distribution for the Higgs mass [4],obtained by convoluting the blue-band plot in Fig.2[1]with the experimental exclusion [3].accompanied by some new physics beyond the Standard Model.The reason for this is to help understand the hi-erarchy of different mass scales in physics,and in par-ticular why m W ≪m P ∼1019GeV ,the Planck mass scale where gravity is expected to become strong and the only candidate we have for a fundamental mass scale in physics.Equivalently,we might ask why there is a hier-archy of different interaction strengths:G F ∼1/m 2W ≫G N =1/m 2P ,or why the Coulomb potential ∼1/r in-side an atom is so much larger than the Newton potential ∼G N m 2=(m/m P )2.You might think one could just ‘set and forget’the mass110101010m H (GeV)S i g n a l s i g n i f i c a n c eFigure 4:The LHC experiments will be able to discover the Higgs boson with high significance,whateveritsmass,and may observe several of its decay modes [6].hierarchy,but then it would be upset by quantum correc-tions.Typical one-loop diagrams in the Standard Model make contributions to the Higgs and W ±masses that di-verge quadratically:δm 2H,W ∼O (απ) m 2B −m 2F ,(4)which would be comparable to m 2W,H if|m 2B −m 2F |∼1TeV 2.(5)This is the motivation for low-energy supersymmetry [7].There is no direct evidence for supersymmetry,but there are several indirect hints that supersymmetry may indeed appear at some energy scale below about 1TeV .One is provided by the strengths of the electromagnetic,weak and strong interactions measured at LEP,which do not ex-trapolate to a common unified value in the absence of su-persymmetry,but do unify at high energies if supersym-metric particles wighing ∼1TeV are included in the renormalization-group equations [8],as seen in Fig.5.An-other hint is provided by the likely mass of the Higgs bo-son.In models with low-energy supersymmetry,it is calcu-lated to weigh less than about 130GeV [9],highly consis-tent with the range suggested by the precision electroweakdata.A third hint may be provided by the dark matter thought to abound in the Universe.The lightest supersym-metric particle (LSP)is stable in the minimal supersymmet-ric extension of the Standard Model (MSSM),and would be an ideal particle candidate for dark matter if it weighs less than about 1TeV [10].Figure 5:The measurements (vertical axis)of the gaugecoupling strengths of the Standard Model at LEP and else-where can be evolved up to high energies (horizontal axis,in units of GeV)using renormalization-group equations in-corporating supersymmetry.They are consistent with uni-fication at a very high energy scale,but not with unification without supersymmetry [8].On the other hand,no sparticles have ever been seen,in particular at LEP,imposing important constraints on the MSSM [11].For example,charginos -the supersymmetric partners of the W ±-must weigh more than about 103GeV ,and slectrons -the supersymmetric partners of the electron -must weigh more than about 100GeV .The lower limit on the mass of the Higgs boson,mentioned above,also imposes an important constraint on the MSSM parameter space,as does the agreement between Standard Model cal-culations and the experimental rate of b →sγdecay,as seen in Fig.6[12].As also seen in Fig.6,a further experimental constraint is provided by the recent measurement of the anomalous magnetic moment of the muon,g µ−2,even if it does not disagree significantly with the Standard Model [13].As things stand,the measured value of g µ−2disagrees by 3standard deviations with the best estimate based on e +e −→hadrons data [14],though the discrepancy with estimates based on τ→hadrons data is less than 2stan-dard deviations.m 0 (G e V )m 1/2 (GeV)Figure 6:The parameter space of the MSSM projected onto the (m 1/2,m 0)plane for tan β=10and µ>0.The LEP lower limits on the Higgs,chargino and selec-tron masses are shown as (red)dot-dashed,(black)dashed and (blue)dash-dotted lines,respectively.The region at small (m 1/2,m 0)excluded by b →sγis shaded (green).The dark (red)shaded region is excluded because dark mat-ter must be neutral,and the region where its relic den-sity falls within the range preferred by cosmology has light (turquoise)shading.The region preferred by the BNL mea-surement of g µ−2and low-energy e +e −data is shaded (pink)[12].5BENCHMARK SUPERSYMMETRICSCENARIOSAs seen in Fig.6,all these constraints on the MSSM are mutually compatible.As an aid to understanding better the physics capabilities of the LHC,various linear e +e −linear collider designs and non-accelerator experiments,a set of benchmark supersymmetric scenarios have been pro-posed [15].These are compatible with all the accelerator constraints mentioned above,including the LEP searches and b →sγ,and yield relic densities of LSPs in the range suggested by cosmology and astrophysics.These bench-marks are not inteneded to sample ‘fairly’the allowed pa-rameter space,but rather to illustrate the range of possibil-ities currently allowed,as shown in Fig.7.In addition to a number of benchmark points falling in the ‘bulk’region of parameter space at relatively low values of the supersymmetric particle masses,we also proposed some points out along the ‘tails’of parameter space extend-ing out to larger masses.These clearly require some de-gree of fine-tuning to obtain the required relic density [16]and/or the correct W ±mass [17],and some are also dis-favoured by the supersymmetric interpretation of the g µ−2anomaly,but all are logically consistent possibilities.Figure 7:Sketch of the distribution of proposed CMSSM benchmark points in the (m 1/2,m 0)plane [15].These points were chosen as illustrations of the range of possi-bilities in the CMSSM,rather than as a ‘fair’sample of its parameter space.6LHC PHYSICSThe cross sections for producing pairs of supersymmet-ric particles at the LHC decrease with increasing masses.Nevertheless,the signature expected for supersymmetry -multiple jets and/or leptons with a large amount of miss-ing energy -is quite distinctive.Therefore,the detection of the supersymmetric partners of quarks and gluons at the LHC is expected to be quite easy if they weigh less than about 2TeV [6].Moreover,in many scenarios one should be able to observe their cascade decays into lighter super-symmetric particles [18].As seen in Fig.8,large fractions of the supersymmetric spectrum should be seen in most of the benchmark scenarios,although there are a couple where only the lightest supersymmetric Higgs boson would be seen [15].N b . o f O b s e r v a b l e P a r t i c l e sFigure 8:Estimates of the numbers of different CMSSM particles that could be seen in each of the benchmark sce-narios [15],at each of the indicated colliders.The points are ordered from left to right by their decreasing compati-bility with the BNL g µ−2measurement.7E +E −LINEAR COLLIDER PHYSICSElectron-positron colliders provide very clean experi-mental environments,with egalitarian production of all the new particles that are kinematically accessible,including those that have only weak interactions.Moreover,po-larized beams provide a useful analysis tool,and eγ,γγand e −e −colliders are readily available at relatively low marginal costs.The e +e −→¯tt threshold is known to be at E CM ∼350GeV .Moreover,if the Higgs boson indeed weighs less than 200GeV ,as suggested by the precision electroweak data,its production and study would also be easy at an e +e −collider with E CM ∼500GeV .With a luminos-ity of 1034cm −2s −1or more,many decaymodes of the Higgs boson could be measured very accurately [19],and one might be able to find a hint whether its properties were modified by supersymmetry [20].However,the direct production of supersymmetric parti-cles at such a collider cannot be guaranteed [21],as seen in Fig.8[15].We do not yet know what the supersymmet-ric threshold energy may be (or even if there is one!).We may well still not know before the operation of the LHC,although g µ−2might provide an indication,if the uncer-tainties in the Standard Model calculation can be reduced.If an e +e −collider is above the supersymmetric thresh-old,it will be able to measure very accurately the sparticle masses.By comparing their masses with those of different sparticles produced at the LHC as seen in Fig.9,one would be able to make interesting tests of string and GUT models of supersymmetry breaking [15].However,independently from the particular benchmark scenarios proposed,a linear e +e −collider with E CM <1TeV would not cover all the supersymmetric parameter space allowed by cosmology.Nevertheless,there are compelling physics arguments for such a linear e +e −collider,which would be very com-plementary to the LHC in terms of its exploratory power and precision.It is to be hoped that the world community will converge on a single project with the widest possible energy range.8CLICCERN and its collaborating institutes are studying the possible following step in linear e +e −colliders,a multi-TeV machine called CLIC [23].This would use a double-beam technique to attain accelerating gradients as high as 150MV/m,and the viability of accelerating structures ca-pable of achieving this field has been demonstrated in the CLIC test facility [24].Parameter sets have been calculated for CLIC designs with E CM =3and 5TeV ,and luminosi-ties of 1035cm −2s −1or more.In many of the proposed benchmark supersymmetric scenarios,CLIC would be able to complete the supersym-metric spectrum and/or measure in much more detail heavy sparticles found previously at the LHC.CLIC produces more beamstrahlung than lower-energy linear e +e −col-liders,but the supersymmetric missing-energy signatureFigure 9:Analogously to the unification of the gauge couplings shown in Fig.5,measurements of the sparticle masses at future colliders (vertical axis,in units of GeV)can be evolved up to high scales (horizontal axis,in units of GeV)to test models of supersymmetry breaking,in par-ticular whether squark and slepton masses are universal at some input GUT scale [22].would still be easy to distinguish,and accurate measure-ments of masses and decay modes could still be made,as seen in Fig.10[25].Smuon Mass (GeV)χ0M a s s (G e V )620640660680700111011201130114011501160117011801190Figure 10:Like lower-energy e +e −colliders,CLIC en-ables very accurate measurements of sparticle masses to be made,in this case the supersymmetric partner of the muon and the lightest neutralino χ0[25].9PERSPECTIVESIn this brief talk,I have tried to explain why higher-energy physics requires higher luminosities and hence smaller beams.I have used the Higgs boson and supersym-metry as examples of the new physics that may be await-ing us at the TeV scale,and shown how they could be ex-plored by colliders with luminosities that are sufficiently high.Other examples,including extra dimensions,are con-sidered in[26].One can already say that linear e+e−col-liders with energies in the sub-and multi-TeV ranges would both be interesting.What ideas exist for colliders to achieve even higher en-ergies?One possibility might be a VLHC with E CM∼100TeV or more.In order to realize its full kinematic potential,such a machine should have a luminosity of 1035cm−2s−1or more,favouring very small beams.As for leptons,e+e−colliders with E CM∼10TeV or more are very difficult to imagine.An alternative might be a very high-energyµ+µ−collider,but this would have to surmount the hurdles of muon cooling and neutrino radi-ation.Even before such futuristic devices,there will be plenty of work for the nanobeam community.10REFERENCES[1]M.W.Grunewald,“Electroweak physics”,arXiv:hep-ex/0210003.[2]P.W.Higgs,Phys.Lett.12(1964)132and Phys.Rev.Lett.13(1964)508;F.Englert and R.Brout,Phys.Rev.Lett.13 (1964)321.[3]LEP Higgs Working Group,http://lephiggs.web.cern.ch/LEPHIGGS/papers/July2002。
