期权期货及其衍生产品约翰赫尔官方课件
合集下载
期权期货及其衍生产品约翰赫尔官方课件

Options, Futures, and Other Derivatives, 8th Edition, Copyright © John C. Hull 2012
12
Theoretical Justification for Cap Model
Working in a world that is FRN wrt a zero - coupon bond maturing at time t k 1 the option price is P (0, t k 1 ) Ek 1[max( Rk RK ,0)] Also Ek 1[ Rk ] Fk This leads to Black's model
where D is the (modified) duration of the forward bond at option maturity
Options, Futures, and Other Derivatives, 8th Edition, Copyright © John C. Hull 2012
Both the bond price and the strike price should be cash prices not quoted prices
Options, Futures, and Other Derivatives, 8th Edition, Copyright © John C. Hull 2012 5
Options, Futures, and Other Derivatives, 8th Edition, Copyright © John C. Hull 2012 13
Swaptions
A swaption or swap option gives the holder the right to enter into an interest rate swap in the future Two kinds
期权期货及其衍生产品约翰赫尔官方课件97522共21页PPT资料

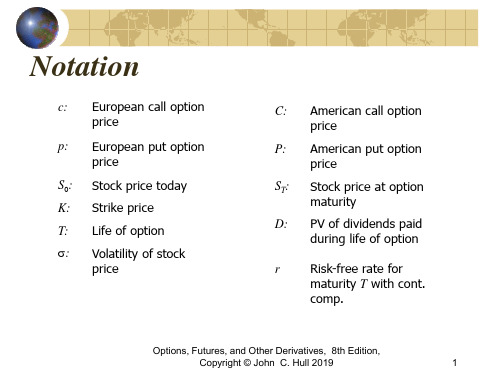
ST: Stock price at option maturity
D: PV of dividends paid during life of option
r Risk-free rate for maturity T with cont. comp.
Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
7
Lower Bound for European Put Prices; No Dividends
(Equation 10.5, page 221)
p Ke -rT–S0
Options, Futures, and Other Derivatives, 8th Edition,
Chapter 10 Properties of Stock Options
Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
1
Notation
c: European call option price
Copyright © John C. Hull 2019
8
Put-Call Parity: No Dividends
Consider the following 2 portfolios: Portfolio A: European call on a stock + zerocoupon bond that pays K at time T Portfolio C: European put on the stock + the stock
D: PV of dividends paid during life of option
r Risk-free rate for maturity T with cont. comp.
Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
7
Lower Bound for European Put Prices; No Dividends
(Equation 10.5, page 221)
p Ke -rT–S0
Options, Futures, and Other Derivatives, 8th Edition,
Chapter 10 Properties of Stock Options
Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
1
Notation
c: European call option price
Copyright © John C. Hull 2019
8
Put-Call Parity: No Dividends
Consider the following 2 portfolios: Portfolio A: European call on a stock + zerocoupon bond that pays K at time T Portfolio C: European put on the stock + the stock
期权期货及其衍生产品约翰赫尔官方课件97522共21页
Notation
c: European call option price
p: European put option price
S0: Stock price today K: Strike price T: Life of option s: Volatility of stock
price
C: American call option price
ST > K ST − K
K ST 0 ST ST
ST < K 0 K K
K− ST ST K
Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
9
The Put-Call Parity Result (Equation
P: American put option price
ST: Stock price at option maturity
D: PV of dividends paid during life of option
r Risk-free rate for maturity T with cont. comp.
(Equation 10.5, page 221)
p Ke -rT–S0
Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
7
Put-Call Parity: No Dividends
Cc Pp
Options, Futures, and Other Derivatives, 8th Edition,
c: European call option price
p: European put option price
S0: Stock price today K: Strike price T: Life of option s: Volatility of stock
price
C: American call option price
ST > K ST − K
K ST 0 ST ST
ST < K 0 K K
K− ST ST K
Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
9
The Put-Call Parity Result (Equation
P: American put option price
ST: Stock price at option maturity
D: PV of dividends paid during life of option
r Risk-free rate for maturity T with cont. comp.
(Equation 10.5, page 221)
p Ke -rT–S0
Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
7
Put-Call Parity: No Dividends
Cc Pp
Options, Futures, and Other Derivatives, 8th Edition,
期权期货及其衍生产品约翰赫尔官方课件共29页

Copyright © John C. Hull 2019
2
Mechanics of Put Futures Option
When a put futures option is exercised the holder acquires
A short position in the futures A cash amount equal to the excess of the strike price over the futures price at the time of the most recent settlement
Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
1
Mechanics of Call Futures Options
When a call futures option is exercised the holder acquires
A long position in the futures A cash amount equal to the excess of the futures price at the time of the most recent settlement over the strike price
Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
6
Potential Advantages of Futures Options over Spot Options
期权期货及其衍生产品约翰赫尔官方课件-PPT精选文档

Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
2
Day Count Conventions in the U.S. (Page 129)
Treasury Bonds: Actual/Actual (in period)
Bond: 8% Actual/ Actual in period.
4% is earned between coupon payment dates. Accruals on an Actual basis. When coupons are paid on March 1 and Sept 1, how much interest is earned between March 1 and April 1?
Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
10
Conversion Factor
Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
4
Examples continued
T-Bill: 8% Actual/360:
8% is earned in 360 days. Accrual calculated by dividing the actual number of days in the period by 360. How much interest is earned between March 1 and April 1?
期权期货及其衍生产品约翰赫尔官方课件97522PPT精品文档21页

Copyright © John C. Hull 2019
2
Effect of Variables on Option Pricing (Table 10.1, page 215)
Variable
c
p
C
P
S0
+
−
+
−
K
−
+
−
+
T
?
?
+
+
s
+
+
+
+
r
+
−
+
Байду номын сангаас
−
D
−
+
−
+
Options, Futures, and Other Derivatives, 8th Edition,
Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
11
Arbitrage Opportunities
Suppose that
c= 3 T = 0.25 K =30
Copyright © John C. Hull 2019
5
Lower Bound for European Call Option Prices; No Dividends (Equation 10.4, page 220)
c S0 –Ke -rT
Options, Futures, and Other Derivatives, 8th Edition,
赫尔《期权期货及其他衍生产品》第1章(第八版)讲述

期权、期货及其他衍生产品(第八版) Copyright © John C. Hull 2012
32
对冲基金( 见业界事例1-2,p8)
• 对冲基金受到的约束与共同基金不同,一般不对外公 布持有的证券组合。
• 共同基金必须
– 披露投资策略 – 在任意时刻允许份额赎回 – 杠杆率受到限制 – 不能持有空头头寸
20
2. 石油:另外一种套利机会?
假定:
- 石油的即期价格为95美元 - 1年期原油期货的标价为80美元 - 1年期的美元利率为 5% - 原油的储存成本为每年2%
是否存在套利机会?
期权、期货及其他衍生产品(第八版) Copyright © John C. Hull 2012
21
期权
• 看涨期权:其持有者有权在将来某一特定时间 以某一确定价格( 执行价格)买入某种资产。
卖出价 1.4411 1.4413 1.4415 1.4422
期权、期货及其他衍生产品(第八版) Copyright © John C. Hull 2012
8
远期价格
• 合约的远期价格是今天约定的合约支付价 格(使合约价值为零的支付价格);
• 对不同期限的远期合约而言,远期价格也 不同(如表1-1所示) 。
期权、期货及其他衍生产品(第八版) Copyright © John C. Hull 2012
34
期权、期货及其他衍生产品(第八版) Copyright © John C. Hull 2012
23
表1-2 谷歌股票看涨期权在2010年6月15日的价格
(P6)
执行 2010年7月
价格
买入价
2010年7月 卖出价
2010年9月 买入价
期权、期货及其他衍生产品第9版-赫尔】Ch(9)幻灯片PPT

Options, Futures, and Other Derivatives, 9th Edition, Copyright ©
John C. Hull 2014
4
Historical Simulation to Calculate the One-Day VaR
Create a database of the daily movements in all market variables. The first simulation trial assumes that the percentage changes in all market variables are as on the first day The second simulation trial assumes that the percentage changes in all market variables are as on the second day and so on
Let vi be the value of a variable on day i
There are 500 simulation trials
The ith trial assumes that the value of the market
variable tomorrow is
v500
vi vi1
期权、期货及其他衍生产品第9 版-赫尔】Ch(9)幻灯片PPT
本PPT课件仅供大家学习使用 请学习完及时删除处理 谢谢!
Options, Futures, and Other Derivatives, 9th Edition,
Copyright © John C. Hull 2014
期权期货及其衍生产品约翰赫尔官方课件

Options, Futures, and Other Derivatives, 8th Edition, Copyright © John C. Hull 2012
9
First Scenario for the Example:
Table 18.2 page 384
Week 0 Stock price 49.00 Delta 0.522 Shares purchased 52,200 Cost (‘$000) 2,557.8 Cumulative Cost ($000) 2,557.8 Interest 2.5
Relationship Between Delta, Gamma, and Theta (page 393)
For a portfolio of derivatives on a stock paying a continuous dividend yield at rate q it follows from the Black-ScholesMerton differential equation that
Options, Futures, and Other Derivatives, 8th Edition, Copyright © John C. Hull 2012
11
Theta
Theta (Q) of a derivative (or portfolio of derivatives) is the rate of change of the value with respect to the passage of time The theta of a call or put is usually negative. This means that, if time passes with the price of the underlying asset and its volatility remaining the same, the value of a long call or put option declines
期权期货及其衍生产品约翰赫尔官方课件

c S0e qT Ke rT
Lower Bound for puts
p Ke
Put Call Parity
rT
S0e
qT
c Ke rT p S0e qT
c Ke rT p F0e rT
11
Options, Futures, and Other Derivatives, 8th Edition, Copyright © John C. Hull 2012
Options, Futures, and Other Derivatives, 8th Edition, Copyright © John C. Hull 2012
5
Example 2
Portfolio has a beta of 2.0 It is currently worth $500,000 and index stands at 1000 The risk-free rate is 12% per annum The dividend yield on both the portfolio and the index is 4% How many put option contracts should be purchased for portfolio insurance?
Options, Futures, and Other Derivatives, 8th Edition, Copyright © John C. Hull 2012 8
European Options on Assets Providing a Known Yield
We get the same probability distribution for the asset price at time T in each of the following cases: 1. The asset starts at price S0 and provides a yield = q 2. The asset starts at price S0e–qT and provides no income
赫尔《期权期货及其他衍生产品》第3章(第八版)

15
为什么要对冲股权组合?
• 如果想暂时离开市场一会儿, 那么对冲可以避免出售资产和购买组合 的成本。
• 假设你投资组合的β均值为1.0。你认为你的投资组合挑选较为完美, 在任何市场情形下都能战胜市场。对冲可以确保你的收益为无风险收 益与投资组合超额收益(相对于市场组合)之和。
期权、期货及其他衍生产品(第八版) Copyright © John C.Hull 2012
• 如果在对冲上有损失、在标的资产上有收益, 如何解释这种情形较为困难。
期权、期货及其他衍生产品(第八版) Copyright © John C.Hull 2012
4
基差风险
• 基差=被对冲风险的即期价格-用于对冲的期货合约的价格 • 对冲被平仓时基差的不确定, 导致了基差风险的产生。
期权、期货及其他衍生产品(第八版) Copyright © • 选择与对冲的到期日最近, 但仍长于对冲到期日的交割月份; • 当被对冲的资产与期货的标的资产不吻合时, 选择期货价格与被对冲
资产的价格相关性最高的期货合约。这就是交叉对冲。
期权、期货及其他衍生产品(第八版) Copyright © John C.Hull 2012
8
最小方差对冲比率的计算(p41)
2
拥护对冲的观点
• 公司应该集中精力发展自身的主要业务, 采取措施将由利率、汇率和 其他市场变量所引起的风险降至最低。
期权、期货及其他衍生产品(第八版) Copyright © John C.Hull 2012
3
反对对冲的观点
• 股东通常持有充分分散的投资组合, 他们可以 自己对冲面临的风险;
• 当竞争对手选择不对冲风险时, 这会导致对冲 风险的上升;
6
期权期货及其衍生产品约翰赫尔官方课件

The value of the portfolio, , is given by ƒ ƒ S S The change in its value in time Dt is given by ƒ D Dƒ DS S
Options, Futures, and Other Derivatives, 8th Edition, Copyright © John C. Hull 2012
10
Nature of Volatility
Volatility is usually much greater when the market is open (i.e. the asset is trading) than when it is closed For this reason time is usually measured in “trading days” not calendar days when options are valued It is assumed that there are 252 trading days in one year for most assets
Options, Futures, and Other Derivatives, 8th Edition, Copyright © John C. Hull 2012
12
The Concepts Underlying BlackScholes-Merton
The option price and the stock price depend on the same underlying source of uncertainty We can form a portfolio consisting of the stock and the option which eliminates this source of uncertainty The portfolio is instantaneously riskless and must instantaneously earn the risk-free rate This leads to the Black-Scholes-Merton differential equation
Options, Futures, and Other Derivatives, 8th Edition, Copyright © John C. Hull 2012
10
Nature of Volatility
Volatility is usually much greater when the market is open (i.e. the asset is trading) than when it is closed For this reason time is usually measured in “trading days” not calendar days when options are valued It is assumed that there are 252 trading days in one year for most assets
Options, Futures, and Other Derivatives, 8th Edition, Copyright © John C. Hull 2012
12
The Concepts Underlying BlackScholes-Merton
The option price and the stock price depend on the same underlying source of uncertainty We can form a portfolio consisting of the stock and the option which eliminates this source of uncertainty The portfolio is instantaneously riskless and must instantaneously earn the risk-free rate This leads to the Black-Scholes-Merton differential equation
期权期货及其衍生产品约翰赫尔官方课件PPT资料29页

Options, Futures, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
4
Example 1 (page 361)
Dec. call option contract on copper futures has a strike of 240 cents per pound. It is exercised when futures price is 251 cents and most recent settlement is 250. One contract is on 250,000 pounds
A long position in the futures A cash amount equal to the excess of the futures price at the time of the most recent settlement over the strike price
Options, Futures, and Other Derivatives, 8th Edition,
If the futures position is closed out immediately: Payoff from call = F– K Payoff from put = K – F where F is futures price at es, and Other Derivatives, 8th Edition,
Copyright © John C. Hull 2019
3
Mechanics of Put Futures Option
When a put futures option is exercised the holder acquires
[考研专业课课件]_赫尔《期货、期权及其他衍生产品》_课件_第22章__估计波动率和相关系数
![[考研专业课课件]_赫尔《期货、期权及其他衍生产品》_课件_第22章__估计波动率和相关系数](https://img.taocdn.com/s3/m/b67624177e21af45b307a8b5.png)
目标是估计当前波动率σn的水平,因此将较大的
权重用在最近的数据更有意义。
模型:
m
2 n
i uni 2
(22-4)
i 1
αi——第i天以前观察值所对应的权重,α
取正值
如果i>j,则αi<αj,将较少的权重给予较
旧的数据。权重之和必须为1,即ARCH(m)模型。
推广式(22-4)
假定存在某一长期平均方差,并且应当给予
σn2——方差率(variance rate)
Si——市场变量在i天末的价格
ui——在第i天连续复利收益率 σn2的无偏估计为
ui
ln
Si Si 1
:
2 n
1 m 1
m i 1
2
uni u
(22-1)
u ——ui的平均值
u
1 m
m
uni
i 1
:
为了监测日方差率的变化:
令w=γVL,可以将式(22-5)写成
m
2 n
i uni 2
(22-6)
i 1
式(22-4)及式(22-5)是后两节中将讨论
的两种测算波动率的重要方法的基础。
22.2 指数加权移动平均模型 指数加权移动平均模型(EWMA)是式(22-4) 的一个特殊形式,其中权重αi随着时间以指数速 度递减,具体地讲,αi+1=λαi,其中λ是介于0 与1之间的某一常数。在以上特殊假设下,更新波 动率公式被简化为
GARCH(1,1)与EWMA模型类似,其不同之处 是除了对过去的u2权重按指数下降的同时,对于 长期平均浮动率赋予了一定的权重。
期货期权及其衍生品配套课件(全34章)Ch03.ppt

Cost of Asset=S2 – (F2 – F1) = F1 + Basis
Options, Futures, and Other Derivatives, 7th International
Edition, Copyright © John C. Hull 2008
7
Short Hedge
Options, Futures, and Other Derivatives, 7th International
Edition, Copyright © John C. Hull 2008
8
Choice of Contract
Choose a delivery month that is as close as possible to, but later than, the end of the life of the hedge When there is no futures contract on the asset being hedged, choose the contract whose futures price is most highly correlated with the asset price. This is known as cross hedging.
Options, Futures, and Other Derivatives, 7th International
Edition, Copyright © John C. Hull 2008
6
Long Hedge
We define
F1 : Initial Futures Price F2 : Final Futures Price S2 : Final Asset Price If you hedge the future purchase of an asset by entering into a long futures contract then
Options, Futures, and Other Derivatives, 7th International
Edition, Copyright © John C. Hull 2008
7
Short Hedge
Options, Futures, and Other Derivatives, 7th International
Edition, Copyright © John C. Hull 2008
8
Choice of Contract
Choose a delivery month that is as close as possible to, but later than, the end of the life of the hedge When there is no futures contract on the asset being hedged, choose the contract whose futures price is most highly correlated with the asset price. This is known as cross hedging.
Options, Futures, and Other Derivatives, 7th International
Edition, Copyright © John C. Hull 2008
6
Long Hedge
We define
F1 : Initial Futures Price F2 : Final Futures Price S2 : Final Asset Price If you hedge the future purchase of an asset by entering into a long futures contract then
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
Options, Futures, and Other Derivatives, 8th Edition, Copyright © John C. Hull 2012
3
Short Selling (continued)
At some stage you must buy the securities so they can be replaced in the account of the client You must pay dividends and other benefits the owner of the securities receives There may be a small fee for borrowing the securities
Is there an arbitrage opportunity?
Options, Futures, and Other Derivatives, 8th Edition, Copyright © John C. Hull 2012
7
Another Arbitrage Opportunity?
Suppose that:
F0 = (S0 – I )erT
where I is the present value of the income during life of forward contract
Options, Futures, and Other Derivatives, 8th Edition, Copyright © John C. Hull 2012
The spot price of nondividend-paying stock is $40 The 3-month forward price is US$39 The 1-year US$ interest rate is 5% per annum
Is there an arbitrage opportunity?
Options, Futures, and Other Derivatives, 8th Edition, Copyright © John C. Hull 2012
12
Valuing a Forward Contract
A forward contract is worth zero (except for bid-offer spread effects) when it is first negotiated Later it may have a positive or negative value Suppose that K is the delivery price and F0 is the forward price for a contract that would be negotiated today
A strong positive correlation between interest rates and the asset price implies the futures price is slightly higher than the forward price A strong negative correlation implies the reverse
Chapter 5 Determination of Forward and Futures Prices
Options, Futures, and Other Derivatives, 8th Edition, Copyright © John C. Hull 2012
1
Consumption vs Investment Assets
F0 = S0 e(r–q )T where q is the average dividend yield on the portfolio represented by the index during life of contract
Options, Futures, and Other Derivatives, 8th Edition, Copyright © John C. Hull 2012
14
Forward vs Futures Prices
When the maturity and asset price are the same, forward and futures prices are usually assumed to be equal. (Eurodollar futures are an exception) When interest rates are uncertain they are, in theory, slightly different:
11
When an Investment Asset Provides a Known Yield (Page 109, equation 5.3)
F0 = S0 e(r–q )T
where q is the average yield during the life of the contract (expressed with continuous compounding)
F0: Futures or forward price today
T: Time until delivery date r: Risk-free interest rate for maturity T
Options, Futures, and Other Derivatives, 8th Edition, Copyright © John C. Hull 2012
Options, Futures, and Other Derivatives, 8th Edition, Copyright © John C. Hull 2012
15
Stock Index (Page 112-114)
Can be viewed as an investment asset paying a dividend yield The futures price and spot price relationship is therefore
Options, Futures, and Other Derivatives, 8th Edition, Copyright © John C. Hull 2012
9
If Short Sales Are Not Possible..
Formula still works for an investment asset because investors who hold the asset will sell it and buy forward contracts when the forward price is too low
the value of a long forward contract, ƒ, is (F0 – K )e–rT the value of a short forward contract is (K – F0 )e–rT
Options, Futures, and Other Derivatives, 8th Edition, Copyright © John C. Hull 2012
Options, Futures, and Other Derivatives, 8th Edition, Copyright © John C. Hull 2012 4
Example
You short 100 shares when the price is $100 and close out the short position three months later when the price is $90 During the three months a dividend of $3 per share is paid What is your profit? What would be your loss if you had bought 100 shares?
Options, Futures, and Other Derivatives, 8th Edition, Copyright © John C. Hull 2012
8
The Forward Price
If the spot price of an investment asset is S0 and the futures price for a contract deliverable in T years is F0, then F0 = S0erT where r is the T-year risk-free rate of interest. In our examples, S0 =40, T=0.25, and r=0.05 so that F0 = 40e0.05×0.25 = 40.50
Options, Futures, and Other Derivatives, 8th Edition, Copyright © John C. Hull 2012
13
Valuing a Forward Contract
Page 109-11
By considering the difference between a contract with delivery price K and a contract with delivery price F0 we can deduce that:
6
An Arbit:
The spot price of a non-dividend-paying stock is $40 The 3-month forward price is $43 The 3-month US$ interest rate is 5% per annum
