Singular Calabi-Yau Manifolds and ADE Classification of CFTs
复几何课程

6) Calabi-Yau的C^2整体估计
1) 复Monge-Ampere 测度和复Monge-Ampere方程的弱解, 3x3学时
2) Bedford-Perron方法和弱解存在性, 2x3学时
3) 容度理论, 2x3学时
4) Kolodziej 内部C^0-估计, 3x3学时
方程中的复Monge-Ampere方程的弱解和正则性理论,复几何中的Hodge分解理论,模空间中的Kodaira-Kuranish形变理论和几何的Gromov-Hausdroff 紧性理论,等等。本门课程,主要介绍一些研究复几何的基本知识, 像有关复分析和方程的位势理论和复Monge-Ampere方程的弱解和正则性理论等, 和目前一些人们非常关心的复几何中有关问题。是门高年级本科生自选的专业课程。
复几何课程详细信息
课程号
00102934
学分
3
英文名称
complex geometry
先修课程
复变函数, 微分几何
中文简介
复几何是门丰富,技术性很强的几何与复分析交叉的数学分支。像经典的黎曼一致化定理,是一维复几何中非常深刻的理论。对高维的复流形, 复几何的研究问题就很广了。好多问题与流形的曲率,代数不变量有关。著名的问题, 有凯勒-爱因斯坦度量的存在性,丘成桐的一致化猜测,与数学物理有关的玄理论,还有各种模空间理论,等等。牵及到的研究工具,有复分析中的位势理论,构造全纯截面的Hormander的 L^2,代数几何中的Hartshorne消奇点理论, Mori的极小模型,
英文简介
Complex geometry is one of important branches in differential geometry with a long research history. As we know, the beautiful Riemann uniformization theorem is a fundamental deep result in Riemannian surface theory. In higher dimensions, the research in Complex geometry becomes more and more rich and hard. There are many interesting problems remaining to study in the future, such as the existence problem of Kaehler-Einstein metrics,
Local pointwise estimates for solutions of the $sigma_2$ curvature equation on 4 manifolds

a r X i v :m a t h /0406027v 1 [m a t h .A P ] 2 J u n 2004Local pointwise estimates for solutions of the σ2curvature equation on 4manifolds Zheng-Chao Han ∗Department of Mathematics Rutgers University 110Frelinghuysen Road Piscataway,NJ 08854zchan@ Abstract The study of the k -th elementary symmetric function of the Weyl-Schouten curvature tensor of a Riemannian metric,the so called σk curvature,has produced many fruitful results in conformal geometry in recent years,especially when the dimension of the underlying manifold is 3or 4.In these studies in conformal geometry,the deforming conformal factor is considered to be a solution of a fully nonlinear elliptic PDE.Important advances have been made in recent years in the understanding of the analytic behavior of solutions of the PDE,including the adaptation of Bernstein type estimates in integral form,global and local derivative estimates,classification of entire solutions and analysis of blowing up solutoins.Most of these results require derivative bounds on the σk curvature.The derivative estimates also require an a priori L ∞bound on the solution.This work provides local L ∞and Harnack estimates for solutions of the σ2curvature equation on 4manifolds,under only L p bounds on the σ2curvature,and the natural assumption of small volume(or total σ2curvature).1Introduction and Statements of the resultsThis paper addresses local L ∞and Harnack estimates for admissible solutions w to eitherσ2(g −1◦A g )=K (x ),(1)orσ2(g−10◦A g)=f(x),(2) where g0is afixed background metric on a4-manifold M4and g=e2w(x)g0is a metric conformal to g0,A g is the the Weyl-Schouten tensor of the metric g,A g=12(n−1)g}=A g− ∇2w−dw⊗dw+1Proposition1.If g=e2w|dx|2is locally conformallyflat,thenkσk(g−1◦A g)=(n−2k)kj=1σk−j(g−1◦A g)2j−1 ∇b w,T k−j(g−1◦A g)a b is the(k−j)-th Newton transform of g−1◦A g:T k−j(g−1◦A g)=k−ji=0(−1)iσk−j−i(g−1◦A g)(g−1◦A g)i.In this theorem all norms and differentiation are instrinsic with g.We remind the reader that T k−j(g−1◦A g)is positive definite for1≤j≤k whenA g∈Γ+k ,see[V2].Also note that when2k=n,σk(g−1◦A g)is a pure divergence,confirming the results of[V1]as mentioned above.In her thesis[G1]Gonzalez also exploits the divergence strucuture ofσk(g−1◦A g).See also[G2,G3].Her work deals with the case2k<n as opposed to our case where2k=n.The case she deals with exhibits somewhat different analytical behavior,as hinted by the extra terms in(4)with definite signs after(n−2k).She does not give an expression such as(4),instead,uses an inductive relation betweenσk(g−1◦A g)andσk−1(g−1◦A g).(4)appears to be based on the same analytical structure as exploited in[G1].Since most of the geometric applications involvingσk deal with the case of k=2in dimension4,we limit ourselves to this case in this paper.For simplicity of presentation, we will take the background metric g0=|dx|2to be theflat metric in a ball in R4.For a general g0,only minor computational changes are needed.In our case,the left hand sides of(2)can be written as divergence in the background metric g0:2σ2(g−10◦A g)=−∂a(M a b∂b w),(5) whereM a b=T1(g−10◦A g)a b+|∇w|2Here and in the remaining of the paper,∇w denotes the gradient of w in the background metric g 0.(5),which follows from (4),is already exploited in [CGY2].It turns out to make sense to discuss a weak notion of admissible solution (subsolution,supersolution)of (1)or (2).An admissible W 2,2solution (subsolution,supersoluton)of(1)or (2)is a w ∈W 2,2(M 4)such that,for a.e.x ,A g (x )∈Γ+2,and the left hand side of(1)or (2)=(≤,≥)the right hand side.In Propositions 2and 3we will consider point-wise upper (lower)bound and weak Harnack inequality for a W 2,2admissible subsolution (supersolution)of (2),respectively.In Theorems 2and 3we provide Harnack inequality for W 2,2admissible solutions of (2).For geometric applications involving solutions of(1),the following estimate under small volume is probably most useful.Theorem 1.Assume 0≤inf B 2R K ≤sup B 2R K <∞.There exist absolute constant ǫ0>0small and C ∗>0depending on (sup B 2R K ) B 2R e 4w dvol g 0such that for anyadmissible solution w of (1)on B 2R ⊂R 4,ifB 2R K (x )e 4w dvol g 0<ǫ0,(6)thensup B R e w ≤C∗ 14,andsup B R e w ≤C ∗inf B Re w .Remark.From the proof it will be clear that the condition sup B 2R K <∞may be relaxed to ||K ||p <∞for some p >1.Then ǫ0depends on p and C ∗also depends on p as well as on R −4/p ||K ||L p (B 2R ) B 2R e 4w dvol g 0.If one is willing to assume sup B 2R K <∞and the smallness of (sup B 2R K ) B 2R e 4w dvol g 0,then the following slightly different,lessgeometric version of Theorem 1,is much easier to prove.Theorem 1′.Assume 0≤inf B 2R K ≤sup B 2R K <∞.There exist absolute constants ǫ1>0small and C ∗>0such that for any admissible solution w of (1)on B 4R ⊂R 4,if(sup B 2R K )B 2Re 4w dvol g 0<ǫ1,thensupB Re w≤C∗ 14, andsup B R e w≤C∗infB Re w.Theorems1and1′are consequences of the following propositions and theorems,which provide pointwise and Harnack estimates for solutions/subsolutions/supersolutions of the fully nonlinear equation(2),and are of independent interest.Proposition2.Assume w is an admissible W2,2subsolution of(2)on B2R⊂R4,and||f||L p(B2R)<∞for some p>1.Then w is bounded from above on B R and for anyβ>0,there existsC=C(p,R4(1−1R4 B2R eβw d vol g013(1−13L p(B2R)),thensupB R(γ+w−m)≤C∗ 1β,(8) 1β≤C∗inf B R(γ+M−w).(9)Here and in the following,the integrals are taken with respect to the measure gener-ated by g0.Proposition3.Assume w is an admissible W2,2supersolution of(2)on B2R⊂R4,and||f||L p(B2R)<∞for some p>1.Then w is bounded from below on B R and for anyβ>0,there existsC=C(p,R4(1−1R4 B2R e−βw d vol g013(1−13L p(B2R)),thensupB R(γ+M−w)≤C∗ 1β,(11)1β≤C∗inf B R(γ+w−m).(12)Remark.As will be seen later in the proofs,the estimates of Proposition3follow from the same scheme of proof as for Proposition2.However,slightly different formulations of the estimates of Proposition3follow easily by the superharmonicity of w,which is a con-sequence of A e2w g∈Γ+2.We formulate them in conjuction with those of Proposition2, because,together,they give the followingTheorem2.Let w be an admissible W2,2solution of(2)on B2R⊂R4,and||f||L p(B2R)<∞for some p>1.Setγ=max(1,R4p)||f||1(ii)if m is a lower bound of w on B2R,then we havesup B R (γ+w−m)≤C∗infB R(γ+w−m).(14)A different formulation of Harnack estimate in terms of e w is given asTheorem3.If w is an admissible solution of(2)on B2R⊂R4,and||f||L p(B2R)<∞for some p>1,then there exists C=C(p,R4(1−1define v j(P)=w j◦ϕj(P)+ln|det(dϕj)|,we havev j(P)−1K(P j)→0in L∞and S4|∇v j|4→0.2.If,furthermore,K(x)is C2and satisfies a non-degeneracy condition∆K(P)=0whenever∇K(P)=0.Then there exists apriori C2,αestimate on w j depending on max K,min K,the C2 norm of K and the modulus of continuity of∇2K.Propositions2,3,Theorems2and3are proved by a Moser iteration scheme.However, the fully nonlinear equations(1)or(2),when regarded as an elliptic equation in w in divergence form,is not uniformly elliptic.Moser iteration procedures have been successfully employed to deal with some non-uniformly elliptic quasilinear equations,see the classic paper[S],and,for general reference,also the monographs[GT,LU].But they do not directly apply to our situation.We establish the Moser iteration scheme by exploiting the special divergence structure in the equation through the following Lemma. Main Lemma.Let G(w)be a nonnegative Lipschitz function of w.If w is an admissible subsolution of(2),we will require G′(w)≥0;and if w is an admissible supersolution of (2),we will require G′(w)≤0.Letη∈C20(B2)be a non-negative cut-offfunction on B2 satisfyingη≡1on B1andη|∇2η| |∇η|2.ThenB2η4|G′(w)||∇w|4 B2η2|G(w)| |∇w|2|∇η|2+|∇w|3η|∇η| + B2η4|G(w)||f|,(16) here and in the following,the integrations are all done with respect to the background metric g0,and we write X Y when there is an absolute constant c>0,depending perhaps only on dimension,such that X≤cY.It turns out that[CGY2]already used an integral estimate like the one in the Main Lemma for a specific f≡0and G(w)=w−¯w,where¯w is the average of w on B2R, although they only used that as a step in the classification of entire solutions and did not pursue the iteration of such integral estimates as done here.Most of the results here were obtained in2002;some were in slightly different formulations and had different proofs.In particular,my orginal formulation and proof of Theorem1,using harmonic approximation,requied some boundary information of the solution.I wish to thankProfessors Chang and Yang for their questioning of my earlier proof,which prompted me tofind the current better proof.I would also like to call attention to[G1,G2,G3], where,as mentioned earlier,M.Gonzalez also exploits the divergence structure ofσk and adapts the Moser iteration scheme.However,other than these two similarities on methods,which were developed independently—we didn’t learn of each other’s work until after we both completed our work and began to report on them,there is no overlap between our work.Our work originated from different motivations and address different situations:this work was motived mainly for applications in apriori estimates such as in Theorem4;while Gonzalez’s work was mostly for studying the size of the singular set of solutions to theσk equations of the type similar to(1)with2k<n and their removability. In fact,one of Gonzalez’s theorems says that,when2k<n,an isolated singularity of theσk Yamabe equation withfinite volume is removable.The corresponding statement in our case does not hold,despite our local L∞estimates under small volume.This can be seen from solutions in my joint work[CHY2]with S.-Y.A.Chang and P.Yang.2Sketch of proofsWe willfirst indicate how Theorem1′follows from Proposition2and Theorem3.Then we will provide a proof for the Main Lemma,which is the basis for all the iteration procedures.Finally we will describe the proofs for Propositions2,3,Theorems2,3,and 1.The proof for Proposition1is in fact quite routine,making use of the transformation formulas such as(3)and the fact that∇a T i(g−1◦A g)a b=0in our situation.Since it is not used essentially in this work,it will be omitted here and will be provided in a future work.Proof of Theorem1′.Here we can take R to be1/2.The general case follows from rescaling.(1)has translation covaraince:if we set w=w+1Thus v (z )≤4for |z |≤r 0/ρ.Note that u 2(x )|dx |2=v 2(z )|dz |2,so thatσ2(g −10◦A v )= K(x 0+ρz )v 4,where A v is the Weyl-Schouten tensor of v 2(z )|dz |2.If r 0/ρ≥1,then v (z )≤4on |z |≤1.The conditions for Proposition 2aresatisfied on |z |≤1.Noting that || K||∞=1.So we apply Proposition 2with R =1/2and p =β=4to obtain an absolute constant C ∗>0such that1=v (0)≤C ∗ |z |≤1v 4(z )dz14≤C ∗ ||K ||∞ B 1e 4w (x )dx1r 0 4 |z |≤r 0r 0 4 |x |≤1u 4(x )dx.Recall that ρu (x 0)=1.So we haver 40u (x 0)4≤C 4∗ |x |≤1u 4(x )dx,from which it follows thatsup |x |≤1(1−|x |)u (x )≤4r 0u (x 0)≤4C ∗|x |≤1u 4(x )dx1p )||f ||L p (B 2R ).An almost identicalverification is carried out in the proof of Theorem 1later.Please refer to that part of the proof.Proof of the Main Lemma.Recall that for g=e2w g0=e2w|dx|2,2σ2(g−10◦A g)=−∂a(M a b∂b w),where|∇w|2M a b=T1(g−10◦A g)a b+2 B2|∇w|4η4G′(w)+ B24η3G(w)M a b∂a w∂bη,if G′≥0;≤1We can integrate by parts to estimate the integralB2η3G(w)M a b∂a w∂bη= B2 ∂a w∂b w−|∇w|2δa b ∂a η3∂bηG(w) −η3G(w)|∇w|2∂b w∂bη= B2 ∂a w∂b w−|∇w|2δa b { 3η2∂aη∂bη+η3∂b aη G(w)++G′(w)η3∂a w∂bη}− B2η3G(w)|∇w|2∂b w∂bη= B2 ∂a w∂b w−|∇w|2δa b 3η2∂aη∂bη+η3∂b aη G(w)− B2η3G(w)|∇w|2∂b w∂bη.Using|η∂b aη| |∇η|2,we conclude the proof of the Main Lemma.Proof of Propositions2and3.[S]serves as a useful guide in the adaptation of Moser’s iteration procedure to our situation.The proof of(7)and(10)is done by plugging in(16) G(w)=e4βw,or more strcitly speaking,truncations of e4βw at large|w|.For simplicity, we will not do the truncation in the test function;Instead,we will demonstrate the estimates apriori by simply plugging G(w)=e4βw in(16).ThenB2η4|G′(w)||∇w|4=4|β| B2η4e4βw|∇w|4(17)=4|β|−3 B2η4|∇eβw|4,B 2η2|G (w )||∇w |2|∇η|2=β−2B 2η2|∇e βw |2e 2βw |∇η|2≤β−2B 2η4|∇eβw |412≤12|β|B 2e 4βw |∇η|4,(18)andB 2η3|G (w )||∇w |3|∇η|=|β|−3B 2η3|∇e βw |3e βw |∇η|≤|β|−3B 2η4|∇eβw |434≤34|β|3B 2e 4βw |∇η|4.(19)Combining the above,we haveB 2|∇(ηe βw )|4 (1+β2)B 2e 4βw (|∇η|4+η4)+|β|3||f ||p ||ηe βw ||44p ′,(20)where p ′=p4p ′=1−θ4.We use interpolation to estimate the last term in (20),||ηe βw ||4p ′,in terms of ||ηe βw ||4and ||ηe βw ||q :||ηe βw ||44p ′≤||ηe βw ||4θ4||ηe βw ||4(1−θ)q,(21)and use the Sobolev inequality to estimate||ηeβw||q.Putting these in(20),we have||∇ ηeβw ||44 (1+β2)||(|∇η|+η)eβw||44+q3(1−θ)|β|3||f||p||ηeβw||4θ4||∇ ηeβw ||4(1−θ)4(1+β2)||(|∇η|+η)eβw||44+θ |β|q1−θ||f||1θ||ηeβw||44+(1−θ)||∇ ηeβw ||44. Thus||∇ ηeβw ||44 |β|q1−θ||f||1θ||ηeβw||44+θ−1(1+β2)||(|∇η|+η)eβw||44,and||ηeβw||4q (|β|q)3/θ||f||1/θp||ηeβw||44+θ−1q3(1+β2)||(|∇η|+η)eβw||44.Since34θ||(|∇η|+η)eβw||4,(22) where M is a constant depending on||f||p and p:M∼[p′||f||p](2p′−1)/4+p′.From(22),one can invoke the Moser iteration procedure to prove(7)and(10).To prove(9)and(11),for instance,wefirst plug G(w)=(γ+M−w)4β−3,β=0,32β4η4|∇(γ+M−w)β|4+12β4η4|∇(γ+M−w)β|4+γ−2η3|∇w|3|G(w)||∇η|=|β|−3η3|∇(γ+M−w)β|3(γ+M−w)β|∇η|≤3ǫ4/34ǫ4(γ+M−w)4β|∇η|4,andη4|G(w)||f|=η4(γ+M−w)4β−3|f|≤γ−3η4|f|(γ+M−w)4β. Choosingǫ>0small(its size can be independent ofβas long as4β−3stays away from0),and treating B2γ−3η4|f|(γ+M−w)4βas in(20)and(21)—noting thatγ= max(1,||f||1/3p),we have|4β−3| B2η4|∇(γ+M−w)β|4 β4 B2(γ+M−w)4β(|∇η|4+η4).(23)To complete the proof of(11),we takeβ>3/4.Then G′(w)≤0,so we have(23)and can use it to carry out the Moser iteration to obtain(11)with the restrictionβ>3 there.But this restriction can be dropped via a device such as Lemma5.1in[Gia]. Lemma5.1in[Gia].Let E(t)be a nonnegative bounded function on0≤T0≤T1. Suppose that for T0≤t<s≤T1we haveE(t)≤θE(s)+A(s−t)−α+Bwithα>0,0≤θ<1and A,B nonnegative constants.Then there exists a constant c=c(α,θ),such that for all T0≤ρ<R≤T1we haveE(ρ)≤c A(R−ρ)−α+BTo complete the proof of(9),we can use(23)withβ<3/4,=0and need to appeal to the John-Nirenberg Theorem.For this purpose,we plug G(w)=(γ+M−w)−3into (16).Similar computations as above lead toB2Rη4|∇log(γ+M−w)|4≤ B2R|∇η|4+γ−3 B2R|f|.(24)The right hand side of(24)has an absolute upper bound,so by the John-Nirenberg Theorem,there exist absolute constantsβ1,C∗>0such that1R4 B2R(γ+M−w)−β1 ≤C∗.(25)Then we can use(23)forβ=−β1<0and carry out the Moser iteration to obtain 1β1≤ C∗inf B R(γ+M−w).(26)(26)and(25)imply(9)forβ=β1.The general case follows from the case forβ=β1, (23)and the Sobolev inequality.The proof for(12)and(8)is done similarly.Proof of Theorem2.(13)follows from(11)and(9).(14)follows from(12)and(8). Proof of Theorem3.This will be completed if we can bridge the gap betwen(7)and (10).Note that since A e2w g∈Γ+2,u=e w satisfies−6∆u=Ru3>0,which implies aBMO estimate for w=ln u by0< −∆u(u−1η2)= |∇ln u|2η2+2η∇ln u·∇η,whereηis a standard cut-offfuntion.SoB R|∇w|2≤4 B R|∇η|2 R2.(27)This can also be done as in(2.17)of[CGY2].Then by the John-Nirenberg Theorem on BMO functions,there exist absolute constantsβ∗>0and C∗>0such that1R4 B2R e−β∗w ≤C∗.This estimate,together with(7)and(10),conclude the proof of Theorem3.Proof of Theorem1.The proof consists of two elements:i.There exists absolute constantǫ0>0such that if w is a solution of(1)and B R K(x)e4w≤ǫ0,thenB R/2|∇w|4≤C,(28)where C depends on(sup BK) B R e4w.Rii.Now treat K(x)e4w as f(x).We can verify that,for p>1,R4(1−1The last term in(29)is estimated by Jensen’s inequality asB Rη4K(x)e4w(w−¯w)≤ B R K(x)e4w ln B R K(x)e5w−¯w −ln B R K(x)e4w≤ B R K(x)e4w ln B R K(x)e5(w−¯w) +4¯w−ln B R K(x)e4w . By the Moser-Trudinger inequality,ln B R K(x)e5(w−¯w) ≤c1 B R|∇w|4+c2+ln(sup K)+ln|B R|,(30) where c1,c2>0are absolute constants.By Jensen’s inequality again,we have1e4¯w≤if B R K(x)e4w≤ǫ0.Supposeǫ0>0is chosen so thatǫ0c1<1,then from the previously cited Lemma5.1in[Gia],we conclude that,for some absolute constantδ>0, Bρ|∇w|4≤δ ǫ0 c2+ln sup K B R e4w −lnǫ0 +CR4(R−ρ)−4 .By takingρ=R/2,we obtain(28).We next use(28)to estimate R4(1−1|B R/2| B R/2e4w 5/4,andR4(1−1inequality.For instance,ln B R K(x)e5(w−¯w) ≤ln ||K||p||e5(w−¯w)||p′1≤(c2+ln|B R|)+ln||K||p.p′Now in choosingǫ0,we have to requireǫ0c1(p′)3<1.The rest of the modifications are straightforward.References[BV]S.Brendle and J.Viaclovsky,A variational characterization forσn/2,to appear in Calc.Var.PDE.[CY3]S.-Y.Chang and P.Yang,The Inequality of Moser and Trudinger and applications to conformal geometry,Comm.Pure and Applied Math.,Vol LVI,no.8,August 2003,pp1135-1150.Special issue dedicated to the memory of Jurgen K.Moser. [CGY1]S.-Y.Chang,M.Gursky and P.Yang,An equation of Monge-Amp`e re type in conformal geometry,and four-manifolds of positive Ricci curvature,Annals of Math.,155(2002),709-787.[CGY2]S.-Y.Chang,M.Gursky and P.Yang,An a priori estimate for a fully nonlinear equation on four-manifolds,Dedicated to the memory of Thomas H.Wolff.J.Anal.Math.87(2002),151–186.[CGY3]S.-Y.Chang,M.Gursky and P.Yang,Entire solutions of a fully nonlinear equation,“Lectures in Partial Differential Equations in honor of Louis Nirenberg’s 75th birthday”,chapter3.International Press,2003.[CHY1]S.-Y.A.Chang,Z.Han,and P.Yang,Apriori estimates for solutions of the prescribedσ2curvature equation on S4,work in preparation.[CHY2]S.-Y.A.Chang,Z.Han,and P.Yang,Classification of singular radial solu-tions of theσk-Yamabe equation on annular domains in R n,preprint.Available at /˜zchan.[CHgY]S.-Y.A.Chang,F.Hang,and P.Yang,On a class of locally conformallyflat manifolds,IMRN,No.4(2004),185-209.[ChW]K.S.Chou and X.-J.Wang,Variational theory for Hessian equations,Comm.Pure Appl.Math.,54(2001),1029-1064.[dG]E.De Giorgi,Sulla differenzabilit`a e l’analiticit`a delle estremali degli integrali mul-tipli regolari,Mem.Accad.Sci.Torino Cl.Sci.Fis.Mat.Natur.(3)3(1957),25-43.[F]H.Fang,Conformally invariant curvatures on LCF manifolds,preprint.[Gia]M.Giaquinta,Introduction to Regularity Theory for Nonlinear Elliptic Systems, Birkh¨a user,1993.[GT]D.Gilbarg and N.Trudinger,Elliptic Partial Differential Equations of Second Order,Second Edition,Springer-Verlag,1983.[G1]M.Gonzalez,Ph.D thesis,Princeton University,2004.[G2]M.Gonzalez,Singular sets of a class of locally conformalyflat manifolds,preprint, 2004.[G3]M.Gonzalez,Removability of singularities for a class of fully non-linear equations, preprint,2004.[GLW]P.Guan,C.S.Lin and G.Wang,Application of The Method of Moving Planes to Conformally Invariant Equations,to appear in Mathematische Zeitschrift.[GVW]P.Guan,J.Viaclovsky and G.Wang,Some properties of the Schouten tensor and applications to conformal geometry,Transactions of American Math.Society, 355(2003),925-933.[GW1]P.Guan and G.Wang,Local estimates for a class of fully nonlinear equations arising from conformal geometry,International Mathematics Research Notices,V.2003,Issue26(2003),1413-1432.[GW2]Pengfei Guan and Guofang Wang,A fully nonlinear conformalflow on locally conformallyflat manifolds,Journal fur die reine und angewandte Mathematik,557 (2003),219-238.[GV1]M.Gursky and J.Viacolvsky,A new variational characterization of three-dimensional space forms,Invent.Math.145(2001),251–278.[GV2]M.Gursky and J.Viacolvsky,Fully nonlinear equations on Riemannian manifolds with negative curvature,Indiana Univ.Math.J.52(2003),no.2,399-420.[GV3]M.Gursky and J.Viacolvsky,A fully nonlinear equation on four-manifolds with positive scalar curvature,to appear in Journal of Differential Geometry.[LU]dyzhenskaya and N.Ural’tseva,Linear and Quasilinear Elliptic Equations, Academic Press,1968.[LL1]Aobing Li and YanYan Li,On some conformally invariant fully nonlinear equa-tions,Comm.Pure Appl.Math.56(2003),1414-1464.[LL2]Aobing Li and YanYan Li,A fully nonlinear version of the Yamabe problem anda Harnack type inequality,arXiv:math.AP/0212031v12Dec2002,11pages.[LL3]Aobing Li and YanYan Li,A Liouville type theorem for some conformally invari-ant fully nonlinear equations,arXiv:math.AP/0212376v130Dec2002,10pages.[LL4]Aobing Li and YanYan Li,A general Liouville type theorem for some conformally invariant fully nonlinear equations,arXiv:math.AP/0301239v121Jan2003,16 pages.[LL5]Aobing Li and YanYan Li,Further results on Liouville type theorems for some conformally invariant fully nonlinear equations,arXiv:math.AP/0301254v122Jan 2003,9pages.[LL6]Aobing Li and YanYan Li,On some conformally invariant fully nonlinear equa-tions,Part II:Liouville,Harnack and Yamabe,preprint,65pages.[M1]J.Moser,A new proof of de Giorgi’s theorem concerning the regularity problem for elliptic differential equations,Comm.Pure Appl.Math.13(1960),457-468.[M2]J.Moser,On Harnack’s theorem for elliptic differential equations,Comm.Pure Appl.Math.14(1961),577-591.[S]J.Serrin,Local behavior of solutions of quasilinear equations,Acta Math.,111 (1964),247-302.[TW1]N.Trudinger and X.J.Wang,Hessian measures.I,Dedicated to Olga Ladyzhen-skaya.Topol.Methods Nonlinear Anal.10(1997),no.2,225–239.[TW2]N.Trudinger and X.J.Wang,Hessian measures.II,Ann.of Math.(2)150 (1999),no.2,579–604.[TW3]N.Trudinger and X.J.Wang,Hessian measures.III,J.Funct.Anal.193(2002), no.1,1–23.[V1]J.Viaclovsky,Conformal geometry,contact geometry,and the calculus of varia-tions,Duke Math.J.101,No.2(2000),283-316.[V2]J.Viaclovsky,Estimates and existence results for some fully nonlinear elliptic equa-tions on Riemannian manifolds,Communications in Analysis and Geometry10 (2002),no.4,815-846.[V3]J.Viaclovsky,Conformally Invariant Monge-Amp`e re Partial Differential Equa-tions:Golbal Solutions,Trans.Amer.Math.Soc.352(2000),no.9,4371–4379.。
研究论著完整目录

研究论著完整目录A. 著作1.中国近现代科技奖励制度(曲安京主编). 济南:山东教育出版社,20042.《二十四史》全译—《唐书历志》. 曲安京,纪志刚,袁敏. 北京:中国人事出版社,20043.《周髀算经》新议. 西安:陕西人民出版社,2002,9月4.中国古代科学技术史纲—数学卷(曲安京主编). 沈阳:辽宁教育出版社,2000,7月5.中国古代数理天文学探析(曲安京、纪志刚、王荣彬). 西安:西北大学出版社,1994,10月B. 西文论文1.Why Mathematics in Ancient China? 数理解析研究所講究錄—数学史の研究,京都:京都大学数理解析研究所,2004,5月2.Perche la matematica nella Cina antica? in Michele Emmer ed.: Matematicae Cultura 2003, Milan: Springer-Verlag, 2003, 205-2173.The Third Approach to the History of Mathematics in China, Proceedings ofthe International Congress of Mathematicians 2002, vol. III, Beijing: Higher Education Press, 2002, 947-958实用文档4.Revisiting An Eighth Century Chinese Table of Tangents, History of OrientalAstronomy, Dordrecht: Kluwer Academic Publishers, 2002, 215-2255.Why Interpolation? Historical Perspectives on East Asian Science,Technology and Medicine, Singapore: Singapore University Press & World Scientific Publishing, 2002, 336-3446.Mathematical Methods in Calendar Making. Storia della Scienza, vol.II,Science in China (Italy), Rome: Enciclopedia Italiana, 2001,153~1557.Responses to Prof. Yabuuti's Work: Studies on Mathematical Astronomy inAncient China, East Asian Science, Technology and Medicine, 18 (2001): 20-238.On Complementary Consecutive Labelings of Octahedron. ArsCombinatoria (Canada), 51(1): 287-294, 19999.Interpolations in Medieval Chinese Mathematical Astronomy. In Y. K. Kimand F. Bray ed. Current Perspectives in the History of Science in East Asia (Korea), Seoul: Seoul National University Press, 1999, 264-277,10.Proof of the Pythagorean Theorem in Zhou Bi Suan Jing, 第七届国际中国科学史会议文集(王渝生主编)(Proceeding of the 7th International Conference on the History of Science in China). 郑州:大象出版社(Zhengzhou: The Elephant Press),pp. 179-192, 199911.Numerical Methods in Medieval Chinese Mathematical Astronomy.西北大实用文档学学报-自然科学版(Journal of Northwest University-Natural Science Edition), 28(2): 99-104, 199812.On Hypotenuse Diagrams in Ancient China. Centaurus(Denmark),39(3):193-210,199713.Bian Gang: A Mathematician of the 9th Century. Historia Scientiarum(Japan), 6(1): 17-30,1996C. 日文论文14.中国の数学史研究:回顧と展望. 数理解析研究所講究錄(1317)—数学史の研究(日本,城地茂译),京都:京都大学数理解析研究所,91-107,2003,5月15.祖冲之は、如何に圓周率π=355/113を得たか?数理解析研究所講究錄(1257)—数学史の研究(日本,城地茂译),京都:京都大学数理解析研究所,163-172,2002,4月16.中国古代におけるる日月食の開始終了時刻の算法と外域の暦法との関係. 数学史研究(日本,大橋由紀夫译), No.164: 1-25,2000,3月17.一行の正接関数表. 数学史研究(大橋由紀夫译), No.153: 18~29, 1997,6月18.《紀元暦》の中の逆関数.数学史研究(日本,大橋由紀夫译), No.150: 13~21,1996,9月D. 部分中文论文实用文档19.东汉到刘宋时期历法五行星会合周期数源. 天文学报,33(1):109-112, 199220.东汉到刘宋时期历法上元积年计算. 天文学报, 32(4):436-439, 199121.《授时历》的白赤道坐标变换法. 自然科学史研究, 22(4): 336-350, 2003,10月22.中国古代日食食差算法的原理.自然科学史研究, 21(2):97-114, 2002,4月23.“消息定数”探析(曲安京,王辉,袁敏). 自然科学史研究, 20(4): 302-311, 2001,10月24.中国古代的九服轨影算法(曲安京,袁敏,王辉). 自然科学史研究,20(1): 13-21,2001,1月25.中国古代数理天文学研究的新进展. 自然科学史研究, 18(3): 277-281, 1999,7月26.宋代太乙历法钩沉. 自然科学史研究,18(1): 69-77, 1999,1月27.《大衍历》晷影差分表的重构. 自然科学史研究,16(3): 233-244, 1997,7月28.中国古代历法中的三次内插法. 自然科学史研究,15(2): 131-143, 199629.王睿至道乾兴乙未四历历元通考. 自然科学史研究, 13(3): 222-235, 199430.李淳风等人盖天说日高公式修正案研究. 自然科学史研究,12(1): 42-51, 199331.唐宋历法演纪上元积年实例及算法分析. 自然科学史研究, 10(4): 315-326, 199132.祖冲之的圆周率π=355/113是如何得出的?. 自然辩证法通讯,24(3):72-77,2002,6月33.中国古代历法与印度及阿拉伯的关系---以日月食起讫算法为例. 自然辩证法通讯,实用文档22(3): 58-68, 2000,6月34.《周髀算经》的盖天说: 别无选择的宇宙结构. 自然辩证法研究,13(8): 37~40,199735.黄道与盖天说的七衡图. 自然辩证法通讯, 16(6): 55-60, 199436.正切函数表在唐代子午线测量中的应用.汉学研究(台湾),16(1): 91-109, 1998,6月37.中国古代历法中的计时制度. 汉学研究,12(2): 157-172, 1994,12月38.太乙数术中的第一部历法.清华学报(台湾),28(2):203-220, 199839.再论刘徽关于阳马与羡除公式的证明. 清华学报(台湾),27(2): 201-215, 199740.商高、赵爽与刘徽关于勾股定理的证明.数学传播(台湾),20(3): 20~27, 199641.唐宋历法中的交食周期与连分数算法. 数学传播(台湾),19(4): 73~79, 199542.《天文大成管窥辑要》中的黄赤道差与白道交周算法. 中国科技史料,16(3): 84~91, 199543.中国数学史家李继闵的生平与成就. 中国科技史料,18(1): 71~79, 199744.再论隋代前后的太阳视运动理论. 大自然探索, 13(3): 104-111, 199445.中国数学史研究的两次运动. 科学,56(2):27-30,200446.刘徽割圆术的数学原理. 刘徽研究(吴文俊主编),170-192. 西安:陕西教育出版社, 199347.试论东汉四分历乾象历景初历上元与五星会合周. 中国天文学史文集, 6: 59-80, 实用文档北京:科学出版社, 199448.唐代太乙数术中的历法探微. 周秦汉唐研究, 1: 381-400, 西安:西北大学出版社,1997.49.宋代易学中的非十进制记数法与贾宪三角形. 周秦汉唐文化研究I, 西安:三秦出版社,102-113,2002,10月50.中国古代对于岁差现象的认识.周秦汉唐文化研究II,西安:三秦出版社,2003,122~14151.中国古代的置闰法:一个概率问题. 西北大学学报-自然科学版, 30(6): 193-195,2000,12月52.《大衍历》定朔算法及程序说明(尚晓清、曲安京). 西北大学学报-自然科学版,29(3): 193-195, 1999 ,6月53.中国古代没灭术算法的意义(曲安京、李彩萍、韩其恒). 西北大学学报-自然科学版, 28(5): 369-373, 199854.中国古代的二次求根公式与反函数. 西北大学学报-自然科学版, 27(1): 1-5,199755.边冈逐次分段抛物插值算法. 西北大学学报-自然科学版,26(1): 1-6, 199656.中国古代历法中之朔望月常数的选择. 西北大学学报-自然科学版,24(4):323-329, 199457.汉历连分数算法质疑. 数学史研究文集(李迪主编), 6: 13-21. 呼和浩特:内蒙古实用文档大学出版社, 199858.论中国古代历法中之闰周的数学性质, 数学史研究文集(李迪主编), 5: 14-25. 呼和浩特:内蒙古大学出版社, 199359.日高图复原, 数学史研究文集(李迪主编), 3: 45-48. 呼和浩特:内蒙古大学出版社, 199260.秦九韶程行相及题意辨析, 数学史研究文集(李迪主编), 2: 71-73. 呼和浩特:内蒙古大学出版社, 199161.大明历上元积年计算. 数学史研究文集(李迪主编),2: 51-57. 呼和浩特:内蒙古大学出版社, 199162.中国古代历法中的上元积年计算. 数学史研究文集(李迪主编), 1: 24-36. 呼和浩特:内蒙古大学出版社, 199063.唐宋时期的“调日法”探微. 纯粹数学与应用数学, 13(专辑):29~34,1997实用文档。
Analysis of some singular solutions in fluid dynamics

ICM 2002 · Vol. III · 1–3
Analysis of Some Singular Solutions in Fluid Dynamics∗
Zhouping Xin†
Abstract
Studies on singular flows in which either the velocity fields or the vorticity fields change dramatically on small regions are of considerable interests in both the mathematical theory and applications. Important examples of such flows include supersonic shock waves, boundary layers, and motions of vortex sheets, whose studies pose many outstanding challenges in both theoretical and numerical analysis. The aim of this talk is to discuss some of the key issues in studying such flows and to present some recent progress. First we deal with a supersonic flow past a perturbed cone, and prove the global existence of a shock wave for the stationary supersonic gas flow past an infinite curved and symmetric cone. For a general perturbed cone, a local existence theory for both steady and unsteady is also established. We then present a result on global existence and uniqueness of weak solutions to the 2-D Prandtl’s system for unsteady boundary layers. Finally, we will discuss some new results on the analysis of the vortex sheets motions which include the existence of 2-D vortex sheets with reflection symmetry; and no energy concentration for steady 3-D axisymmetric vortex sheets.
黎曼几何教学简介
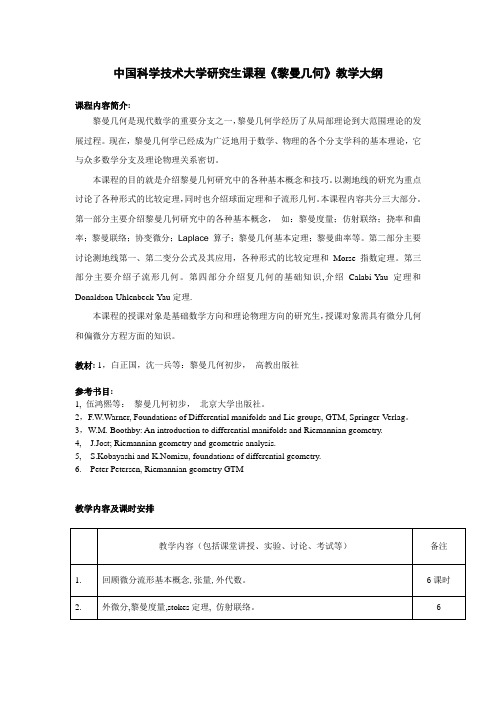
中国科学技术大学研究生课程《黎曼几何》教学大纲课程内容简介:黎曼几何是现代数学的重要分支之一,黎曼几何学经历了从局部理论到大范围理论的发展过程。
现在,黎曼几何学已经成为广泛地用于数学、物理的各个分支学科的基本理论,它与众多数学分支及理论物理关系密切。
本课程的目的就是介绍黎曼几何研究中的各种基本概念和技巧。
以测地线的研究为重点讨论了各种形式的比较定理,同时也介绍球面定理和子流形几何。
本课程内容共分三大部分。
第一部分主要介绍黎曼几何研究中的各种基本概念,如:黎曼度量;仿射联络;挠率和曲率;黎曼联络;协变微分;Laplace 算子;黎曼几何基本定理;黎曼曲率等。
第二部分主要讨论测地线第一、第二变分公式及其应用,各种形式的比较定理和Morse指数定理。
第三部分主要介绍子流形几何。
第四部分介绍复几何的基础知识,介绍Calabi-Yau定理和Donaldson-Uhlenbeck-Yau定理.本课程的授课对象是基础数学方向和理论物理方向的研究生,授课对象需具有微分几何和偏微分方程方面的知识。
教材: 1,白正国,沈一兵等:黎曼几何初步,高教出版社参考书目:1, 伍鸿熙等:黎曼几何初步,北京大学出版社。
2,F.W.Warner, Foundations of Differential manifolds and Lie groups, GTM, Springer-Verlag。
3,W.M. Boothby: An introduction to differential manifolds and Riemannian geometry.4, J.Jost; Riemannian geometry and geometric analysis.5, S.Kobayashi and K.Nomizu, foundations of differential geometry.6. Peter Petersen, Riemannian geometry GTM教学内容及课时安排。
漫谈微分几何、多复变函数与代数几何(Differential geometry, functions

漫谈微分几何、多复变函数与代数几何(Differential geometry, functions of complex variable and algebraic geometry)Differential geometry and tensor analysis, developed with the development of differential geometry, are the basic tools for mastering general relativity. Because general relativity's success, to always obscure differential geometry has become one of the central discipline of mathematics.Since the invention of differential calculus, the birth of differential geometry was born. But the work of Euler, Clairaut and Monge really made differential geometry an independent discipline. In the work of geodesy, Euler has gradually obtained important research, and obtained the famous Euler formula for the calculation of normal curvature. The Clairaut curve of the curvature and torsion, Monge published "analysis is applied to the geometry of the loose leaf paper", the important properties of curves and surfaces are represented by differential equations, which makes the development of classical differential geometry to reach a peak. Gauss in the study of geodesic, through complicated calculation, in 1827 found two main curvature surfaces and its product in the periphery of the Euclidean shape of the space not only depends on its first fundamental form, the result is Gauss proudly called the wonderful theorem, created from the intrinsic geometry. The free surface of space from the periphery, the surface itself as a space to study. In 1854, Riemann made the hypothesis about geometric foundation, and extended the intrinsic geometry of Gauss in 2 dimensional curved surface, thus developing n-dimensional Riemann geometry, with the development of complex functions. A group of excellentmathematicians extended the research objects of differential geometry to complex manifolds and extended them to the complex analytic space theory including singularities. Each step of differential geometry faces not only the deepening of knowledge, but also the continuous expansion of the field of knowledge. Here, differential geometry and complex functions, Lie group theory, algebraic geometry, and PDE all interact profoundly with one another. Mathematics is constantly dividing and blending with each other.By shining the charming glory and the differential geometric function theory of several complex variables, unit circle and the upper half plane (the two conformal mapping establishment) defined on Poincare metric, complex function theory and the differential geometric relationships can be seen distinctly. Poincare metric is conformal invariant. The famous Schwarz theorem can be explained as follows: the Poincare metric on the unit circle does not increase under analytic mapping; if and only if the mapping is a fractional linear transformation, the Poincare metric does not change Poincare. Applying the hyperbolic geometry of Poincare metric, we can easily prove the famous Picard theorem. The proof of Picard theorem to modular function theory is hard to use, if using the differential geometric point of view, can also be in a very simple way to prove. Differential geometry permeates deep into the theory of complex functions. In the theory of multiple complex functions, the curvature of the real differential geometry and other series of calculations are followed by the analysis of the region definition metric of the complex affine space. In complex situations, all of the singular discrete distribution, and in more complex situations, because of the famous Hartogsdevelopment phenomenon, all isolated singularities are engulfed by a continuous region even in singularity formation is often destroyed, only the formation of real codimension 1 manifold can avoid the bad luck. But even this situation requires other restrictions to ensure safety". The singular properties of singularities in the theory of functions of complex functions make them destined to be manifolds. In 1922, Bergman introduced the famous Bergman kernel function, the more complex function or Weyl said its era, in addition to the famous Hartogs, Poincare, Levi of Cousin and several predecessors almost no substantive progress, injected a dynamic Bergman work will undoubtedly give this dead area. In many complex function domains in the Bergman metric metric in the one-dimensional case is the unit circle and Poincare on the upper half plane of the Poincare, which doomed the importance of the work of Bergman.The basic object of algebraic geometry is the properties of the common zeros (algebraic families) of any dimension, affine space, or algebraic equations of a projective space (defined equations),The definitions of algebraic clusters, the coefficients of equations, and the domains in which the points of an algebraic cluster are located are called base domains. An irreducible algebraic variety is a finite sub extension of its base domain. In our numerical domain, the linear space is the extension of the base field in the number field, and the dimension of the linear space is the number of the expansion. From this point of view, algebraic geometry can be viewed as a study of finite extension fields. The properties of algebraic clusters areclosely related to their base domains. The algebraic domain of complex affine space or complex projective space, the research process is not only a large number of concepts and differential geometry and complex function theory and applied to a large number of coincidence, the similar tools in the process of research. Every step of the complex manifold and the complex analytic space has the same influence on these subjects. Many masters in related fields, although they seem to study only one field, have consequences for other areas. For example: the Lerey study of algebraic topology that it has little effect on layer, in algebraic topology, but because of Serre, Weil and H? Cartan (E? Cartan, eldest son) introduction, has a profound impact on algebraic geometry and complex function theory. Chern studies the categories of Hermite spaces, but it also affects algebraic geometry, differential geometry and complex functions. Hironaka studies the singular point resolution in algebraic geometry, but the modification of complex manifold to complex analytic space and blow up affect the theory of complex analytic space. Yau proves that the Calabi conjecture not only affects algebraic geometry and differential geometry, but also affects classical general relativity. At the same time, we can see the important position of nonlinear ordinary differential equations and partial differential equations in differential geometry. Cartan study of symmetric Riemann space, the classification theorem is important, given 1, 2 and 3 dimensional space of a Homogeneous Bounded Domain complete classification, prove that they are all homogeneous symmetric domains at the same time, he guessed: This is also true in the n-dimensional equivalent relation. In 1959, Piatetski-Shapiro has two counterexample and find the domain theory of automorphic function study in symmetry, in the 4 and 5dimensional cases each find a homogeneous bounded domain, which is not a homogeneous symmetric domain, the domain he named Siegel domain, to commemorate the profound work on Siegel in 1943 of automorphic function. The results of Piatetski-Shapiro has profound impact on the theory of complex variable functions and automorphic function theory, and have a profound impact on the symmetry space theory and a series of topics. As we know, Cartan transforms the study of symmetric spaces into the study of Lie groups and Lie algebras, which is directly influenced by Klein and greatly develops the initial idea of Klein. Then it is Cartan developed the concept of Levi-Civita connection, the development of differential geometry in general contact theory, isomorphic mapping through tangent space at each point on the manifold, realize the dream of Klein and greatly promote the development of differential geometry. Cartan is the same, and concluded that the importance of the research in the holonomy manifold twists and turns, finally after his death in thirty years has proved to be correct. Here, we see the vast beauty of differential geometry.As we know, geodesic ties are associated with ODE (ordinary differential equations), minimal surfaces and high dimensional submanifolds are associated with PDE (partial differential equations). These equations are nonlinear equations, so they have high requirements for analysis. Complex PDE and complex analysis the relationship between Cauchy-Riemann equations coupling the famous function theory, in the complex case, the Cauchy- Riemann equations not only deepen the unprecedented contact and the qualitative super Cauchy-Riemann equations (the number of variables is greater than the number of equations) led to a strange phenomenon. This makes PDE and the theory ofmultiple complex functions closely integrated with differential geometry.Most of the scholars have been studying the differential geometry of the intrinsic geometry of the Gauss and Riemann extremely deep stun, by Cartan's method of moving frames is beautiful and concise dumping, by Chern's theory of characteristic classes of the broad and profound admiration, Yau deep exquisite geometric analysis skills to deter.When the young Chern faced the whole differentiation, he said he was like a mountain facing the shining golden light, but he couldn't reach the summit at one time. But then he was cast as a master in this field before Hopf and Weil.If the differential geometry Cartan development to gradually change the general relativistic geometric model, then the differential geometry of Chern et al not only affect the continuation of Cartan and to promote the development of fiber bundle in the form of gauge field theory. Differential geometry is still closely bound up with physics as in the age of Einstein and continues to acquire research topics from physicsWhy does the three-dimensional sphere not give flatness gauge, but can give conformal flatness gauge? Because 3D balls and other dimension as the ball to establish flat space isometric mapping, so it is impossible to establish a flatness gauge; and n-dimensional balls are usually single curvature space, thus can establish a conformal flat metric. In differential geometry, isometry means that the distance between the points on the manifold before and after the mapping remains the same. Whena manifold is equidistant from a flat space, the curvature of its Riemann cross section is always zero. Since the curvature of all spheres is positive constant, the n-dimensional sphere and other manifolds whose sectional curvature is nonzero can not be assigned to local flatness gauge.But there are locally conformally flat manifolds for this concept, two gauge G and G, if G=exp{is called G, P}? G between a and G transform is a conformal transformation. Weyl conformal curvature tensor remains unchanged under conformal transformation. It is a tensor field of (1,3) type on a manifold. When the Weyl conformal curvature tensor is zero, the curvature tensor of the manifold can be represented by the Ricci curvature tensor and the scalar curvature, so Penrose always emphasizes the curvature =Ricci+Weyl.The metric tensor g of an n-dimensional Riemann manifold is conformally equivalent to the flatness gauge locally, and is called conformally flat manifold. All Manifolds (constant curvature manifolds) whose curvature is constant are conformally flat, so they can be given conformal conformal metric. And all dimensions of the sphere (including thethree-dimensional sphere) are manifold of constant curvature, so they must be given conformal conformal metric. Conversely, conformally flat manifolds are not necessarily manifolds of constant curvature. But a wonderful result related to Einstein manifolds can make up for this regret: conformally conformally Einstein manifolds over 3 dimensions must be manifolds of constant curvature. That is to say, if we want conformally conformally flat manifolds to be manifolds of constant curvature, we must call Ric= lambda g, and this is thedefinition of Einstein manifolds. In the formula, Ric is the Ricci curvature tensor, G is the metric tensor, and lambda is constant. The scalar curvature S=m of Einstein manifolds is constant. Moreover, if S is nonzero, there is no nonzero parallel tangent vector field over it. Einstein introduction of the cosmological constant. So he missed the great achievements that the expansion of the universe, so Hubble is successful in the official career; but the vacuum gravitational field equation of cosmological term with had a Einstein manifold, which provides a new stage for mathematicians wit.For the 3 dimensional connected Einstein manifold, even if does not require the conformal flat, it is also the automatic constant curvature manifolds, other dimensions do not set up this wonderful nature, I only know that this is the tensor analysis summer learning, the feeling is a kind of enjoyment. The sectional curvature in the real manifold is different from the curvature of the Holomorphic cross section in the Kahler manifold, and thus produces different results. If the curvature of holomorphic section is constant, the Ricci curvature of the manifold must be constant, so it must be Einstein manifold, called Kahler- Einstein manifold, Kahler. Kahler manifolds are Kahler- Einstein manifolds, if and only if they are Riemann manifolds, Einstein manifolds. N dimensional complex vector space, complex projective space, complex torus and complex hyperbolic space are Kahler- and Einstein manifolds. The study of Kahler-Einstein manifolds becomes the intellectual enjoyment of geometer.Let's go back to an important result of isometric mapping.In this paper, we consider the isometric mapping between M and N and the mapping of the cut space between the two Riemann manifolds, take P at any point on M, and select two non tangent tangent vectors in its tangent space, and obtain its sectional curvature. In the mapping, the two tangent vectors on the P point and its tangent space are transformed into two other tangent vectors under the mapping, and the sectional curvature of the vector is also obtained. If the mapping is isometric mapping, then the curvature of the two cross sections is equal. Or, to be vague, isometric mapping does not change the curvature of the section.Conversely, if the arbitrary points are set, the curvature of the section does not change in nature, then the mapping is not isometric mapping The answer was No. Even in thethree-dimensional Euclidean space on the surface can not set up this property. In some cases, the limit of the geodesic line must be added, and the properties of the Jacobi field can be used to do so. This is the famous Cartan isometry theorem. This theorem is a wonderful application of the Jacobi field. Its wide range of promotion is made by Ambrose and Hicks, known as the Cartan-Ambrose-Hicks theorem.Differential geometry is full of infinite charm. We classify pseudo-Riemannian spaces by using Weyl conformal curvature tensor, which can be classified by Ricci curvature tensor, or classified into 9 types by Bianchi. And these things are all can be attributed to the study of differential geometry, this distant view Riemann and slightly closer to the Klein point of the perfect combination, it can be seen that the great wisdom Cartan, here you can see the profound influence of Einstein.From the Hermite symmetry space to the Kahler-Hodge manifold, differential geometry is not only closely linked with the Lie group, but also connected with algebra, geometry and topologyThink of the great 1895 Poicare wrote the great "position analysis" was founded combination topology unabashedly said differential geometry in high dimensional space is of little importance to this subject, he said: "the home has beautiful scenery, where Xuyuan for." (Chern) topology is the beauty of the home. Why do you have to work hard to compute the curvature of surfaces or even manifolds of high dimensions? But this versatile mathematician is wrong, but we can not say that the mathematical genius no major contribution to differential geometry? Can not. Let's see today's close relation between differential geometry and topology, we'll see. When is a closed form the proper form? The inverse of the Poicare lemma in the region of the homotopy point (the single connected region) tells us that it is automatically established. In the non simply connected region is de famous Rham theorem tells us how to set up, that is the integral differential form in all closed on zero.Even in the field of differential geometry ignored by Poicare, he is still in a casual way deeply affected by the subject, or rather is affecting the whole mathematics.The nature of any discipline that seeks to be generalized after its creation, as is differential geometry. From the curvature, Euclidean curvature of space straight to zero, geometry extended to normal curvature number (narrow Riemann space) andnegative constant space (Lobachevskii space), we know that the greatness of non Euclidean geometry is that it not only independent of the fifth postulate and other alternative to the new geometry. It can be the founder of triangle analysis on it. But the famous mathematician Milnor said that before differential geometry went into non Euclidean geometry, non Euclidean geometry was only the torso with no hands and no feet. The non Euclidean geometry is born only when the curvature is computed uniformly after the metric is defined. In his speech in 1854, Riemann wrote only one formula: that is, this formula unifies the positive curvature, negative curvature and zero curvature geometry. Most people think that the formula for "Riemann" is based on intuition. In fact, later people found the draft paper that he used to calculate the formula. Only then did he realize that talent should be diligent. Riemann has explored the curvature of manifolds of arbitrary curvature of any dimension, but the quantitative calculations go beyond the mathematical tools of that time, and he can only write the unified formula for manifolds of constant curvature. But we know,Even today, this result is still important, differential geometry "comparison theorem" a multitude of names are in constant curvature manifolds for comparison model.When Riemann had considered two differential forms the root of two, this is what we are familiar with the Riemann metric Riemannnian, derived from geometry, he specifically mentioned another case, is the root of four four differential forms (equivalent to four yuan product and four times square). This is the contact and the difference between the two. But he saidthat for this situation and the previous case, the study does not require substantially different methods. It also says that such studies are time consuming and that new insights cannot be added to space, and the results of calculations lack geometric meaning. So Riemann studied only what is now called Riemann metric. Why are future generations of Finsler interested in promoting the Riemann's not wanting to study? It may be that mathematicians are so good that they become a hobby. Cartan in Finsler geometry made efforts, but the effect was little, Chern on the geometric really high hopes also developed some achievements. But I still and general view on the international consensus, that is the Finsler geometry bleak. This is also the essential reason of Finsler geometry has been unable to enter the mainstream of differential geometry, it no beautiful properties really worth geometers to struggle, also do not have what big application value. Later K- exhibition space, Cartan space will not become mainstream, although they are the extension of Riemannnian geometry, but did not get what the big development.In fact, sometimes the promotion of things to get new content is not much, differential geometry is the same, not the object of study, the more ordinary the better, but should be appropriate to the special good. For example, in Riemann manifold, homogeneous Riemann manifold is more special, beautiful nature, homogeneous Riemann manifolds, symmetric Riemann manifold is more special, so nature more beautiful. This is from the analysis of manifold Lie group action angle.From the point of view of metric, the complex structure is given on the even dimensional Riemann manifold, and the complexmanifold is very elegant. Near complex manifolds are complex manifolds only when the near complex structure is integrable. The complex manifold must be orientable, because it is easy to find that its Jacobian must be nonnegative, whereas the real manifold does not have this property in general. To narrow the scope of the Kahler manifold has more good properties, all complex Submanifolds of Kahler manifolds are Kahler manifolds, and minimal submanifolds (Wirtinger theorem), the beautiful results captured the hearts of many differential geometry and algebraic geometry, because other more general manifolds do not set up this beautiful results. If the first Chern number of a three-dimensional Kahler manifold is zero, the Calabi-Yau manifold can be obtained, which is a very interesting manifold for theoretical physicists. The manifold of mirrors of Calabi-Yau manifolds is also a common subject of differential geometry in algebraic geometry. The popular Hodge structure is a subject of endless appeal.Differential geometry, an endless topic. Just as algebraic geometry requires double - rational equivalence as a luxury, differential geometry requires isometric transformations to be difficult. Taxonomy is an eternal subject of mathematics. In group theory, there are single group classification, multi complex function theory, regional classification, algebraic geometry in the classification of algebraic clusters, differential geometry is also classified.The hard question has led to a dash of young geometry and old scholars, and the prospect of differential geometry is very bright.。
武林秘籍与代数几何

武林秘籍与代数几何这篇文章的作者是位在Stanford念代数几何的博士,网名Quillen。
引至博士家园。
笔者是代数几何工作者..认为代数几何比微分几何有趣得多. 虽然微分几何的重要性是无庸置疑,但是代数几何有更多巧妙得构思,也有更有趣的问题.. 让我来说几本代数几何的好书: ( 在书号后是金庸小说密籍的类比,书评之后有两个星号数.第一个是困难度.第二个是重要(趣味)性, 第三个是读了投资报酬率从1 到5 是易到难(无聊到有趣) .. )1 武当长拳( 基本功夫) Atiyah&McDonald 的Introduction to Commutative Algebra和Matsumura 的Commutative Algebra 是代数几何中代数部份的背景知识. 两本书只重视代数而不提及几何,但第一本书的习题有很多引出几何背后意义的好问题. 事实上任何一个交换代数的定理都有几何意义. A&M 的书写的很短, 但是把所有的内容都做了简介, Matsumura 的书内容非常丰富,如果念完她就可以开始交换代数的现代研究,可以开始看文章,这本书比A&M 多了一些重要的章节如"flatness" 和"Struture 定理".-----------------------------困难度中趣味性***2 梯云纵(练了想进哪个分支都可以...) Robin Hartshorne 的Algebraic Geometry是代数几何的经典教科书.任何一个年纪不到五十的代数几何学家都是学这本书长大的.这本书是Grothendick 的EGA 和SGA 一部分的一个非常有系统的总结. Grothendick的书包含的内容很齐全但是失于不实际: 也就是讨论的对象过于一般有时没有几何意义, 这一点十分不好. 但是Hartshorne 的书把整个Grothendick 的Scheme 纲领作了一个最恰当的诠释.这本书的习题也非常重要不管将来对算数几何或复几何或更深入的代数几何这本书的习题都是永远有用的.本书的菁华在前三章,很好的处理了scheme的基础性质,最重要的大定理是第三章的最后一节"上同调与基转换" 定理, 是一个来自复几何的定理. 四五章分别是曲线和曲面, 但是这两个专题都有更好的专书介绍.-----------------------------困难度中等趣味性***3 一套武术服饰(行走江湖要穿衣服)Gunning 的Lectures on Riemann surface 或Forster 或Farkas 或Jost 的Riemann Surface: 黎曼曲面是数学的核心. 跟一切的数学分支都有重大关系. 上述四个作者的书都有相当深度. 笔者只念过Gunning 的, 是一本比较重视"上同调群" 的好书. 其他几本又或重视黎曼面的"双曲几何" 或"黎曼曲面的自同构" 或"曲线上的特殊线性系", 都非常有意思. 很多中国人还喜欢伍鸿禧写的黎曼曲面引论. 但笔者并不是非常喜欢. Gunning 书的优点是把层的上同调做了很快但很详尽的介绍,该书证明Serre对偶定理和Riemann Roch 定理的方法使用了广义函数,和一般的证明不大一样,适合喜欢广义函数多过椭圆方程的读者.------------------------------困难度易趣味性***4 全真派基本内功(一定要练) Griffith& Haris 的Principles inAlgebraic Geometry. 这本书是经典中的经典.是复几何的基本教材. 这本书的每一章都写的很完美. 第一章是Hodge 理论..是复几何中最深奥的理论. 第二章是Kodaira 嵌入定理复流形的嵌入比实流形的嵌入有趣很多. 第三章是current 和spectral sequence, 是很现代的工具. 第四章是曲面论. 写的很详尽但是有更好的书(见6).第五张是特殊专题对袋鼠几何中不同方向的人有不同功用.这本书是学习复几何的必备教材.但是学袋鼠几何的人如果读了这本书,却能对袋鼠几何有一个更全盘更清晰的认识.也就是所谓站在更高的角度.-------------------------------困难度中等趣味性**** 投资报酬率****5 九阳神功Barth & Hulek & Peters 的Compact complex surfaces. 这本书是经典中的经典中的经典. 讲的是代数曲面的各种专题. 每个章节都写的无限完美. 可以说如果学代数几何没念过这本书. 甚至是学几何没念过这本书..可以考虑换行.是百年难得一见的好书. 内容包括曲面里的曲线,相交数,霍奇分解,pojectivity,有理曲面分类,Kodaira分类,general 曲面,K3&Enrique曲面. 笔者以为此书新版的最后两张写的尤其好. 一是K3 曲面另一个是Doanaldson 和Seiber Witten 理论. 后者是来自模空间的不变量理论.现在都是热门的专题.------------------------------困难度中等趣味性***** 投资报酬率*****6 少林派罗汉拳(如果没事可以练练) Robert Friedman 的Algebraic Surfaces and Holomorphic Vector Bundles 这本书是讲曲面和上面的向量丛. 曲面的部分讲得有点乱,事实上没有人把曲面讲的比Barth 还好的. 向量丛的部分有"稳定性条件"的介绍和刻画,值得一看.--------------------------------困难度易投资报酬率***7 双手互博(可以连结两样功夫) William Fulton 的Intersection Theory 相交理论是代数几何1960-1990发展的一套基本理论,阅读很多的专门书籍都需要用到他,本书是相交理论的大家Fulton 的代表作, 介绍了Chow Group 的性质,代数陈省身类, 还有Fulton 发现的deformation to normal cone, 用它来做子簇的香蕉理论,还有很多专题,这些专题都很现代,相交理论是Gromov Witten 不变量,Donaldson 不变量,模空间理论等的基本知识, 基于这些不变量和模空间是现代袋鼠几何的发展潮流, 这本书前六章的必读性并不亚于Griffith & Harris 或是Hartshorne 的书.------------------- 困难舵一点点难, 趣味性***(主要趣味在应用) 报酬率*****8 吸星大法(练完就可以吸取微分拓墣学家的内功以为己用) Donaldson & Kroheimer 的The Geometry of Four manifold. 这是微分拓墣中的圣经.两人都是大家. 此书引出了四维流形的Gauge Invariant (规范不变量), Donaldson因为他在此书的工作,对四维流行的微分结构增加了了解,因而获得菲尔兹奖,而复曲面是四维流形中的一大类..因此也属于代数几何. 现代做这个领域的人不多,但是却是将来几盒和拓墣发展的重大方向,Aityah 曾说"21世纪的数学是规范理论的世纪".--------------------------------困难度难趣味性***** 投资报酬率0 (本书效益在五十年后)9 乾坤大挪移(练到一半就够强了全部练完你也吐血而亡) John Morgan 和Robert Friedman 的Smooth four manifold and Complex surfaces. 这本书讲得是椭圆曲面和其上Donaldson 规范不变量理论.作者利用此理论得到了曲面的一个大定理, 证明了最多只能有有限个复变形类共用一个微分结构. 是一本很专门的书, 内容非常紧凑而且很不容易念,笔者还在努力学习.--------------------------- 困难度极难趣味性**** 投资报酬率**10 Kashiwara的Sheaves on manifolds 这本书非常厚,写的相关层的拓扑性质,有Riemann-Hilbert correspondence, 各种层, 变态层和可建构函数, 笔者没有念过所以无法做更多介绍.11 Hartshorne 的Residues and Dualities 介绍Derived category 和其上的?#092;算,一些对偶定理.和Kashiwara 的书有内容上的重叠,因为Kontsevich 的Homological Mirror Symmetry , 所谓的Derived Category逐渐受到大家的重视.对直攻现代研究有帮助.12 筋肉人和加菲猫的无敌风火轮(练前请三思) Haris 的The Geometry of Algebraic Curves. 是有一点点狭窄的领域. 研究代数曲线上的特殊线性系统. 有很多细节的一本书. 念完后的最大用处就是研究曲线的模空间Mg, 是现在最热门的专题,但是做的人非常多,所以可能入手会很艰辛.也就是很有可能找到你作的题目有其他的大头也一起在做.不论如何,念完此书可以成为一个代数曲线的专家.将来的发展也不少.这个Mg的延续就是Gromov Witten 不变量,以及所谓的保角场论中的\sigma 模型. (来自弦论)------------------- 困难度难趣味性** 投资报酬率***13 五岳派剑法(有用处但是相当杂乱.拼拼凑凑) Joe Harris & David Morrison 的Moduli of Curves 是讲曲线的模空间的经典.但笔者念的有一点头昏脑胀. 这本书的原是前一本书的第二册.也就是研究Mg (亏格g的曲线的模空间)的入门书.里面有Enumerative Geometry (记数几何) 的一个全面介绍. 有曲线模空间上的相交数和各种性质. 该书写的相当有几何风味,至少是Harris 的几何风味.------------- 困难度中等趣味性*** 投资报酬率*****14 九阴真经(练完后可以开始真正研究问题)John Morgan 和Robert Friedman 的Gauge Theory and the Topology of Four-Manifolds. 里面有Gieseker 写几何不变量理论. 李骏的Uhlenbeck 紧化和Gesieker紧化的比较定理. Morgan 讨论Donaldson 规范不变量和对此量的计算结果. 此书的分量不多,也没有太多繁琐的性质.各章都直接介绍最重要的结果和想法.不要求太多细节的验证.-------------------- 困难度中等趣味性***** 投资报酬率*****15 太极拳(一法通万法通) Daniel Huybrecht 的The Geoemtry of Moduli Space of Sheaves. 是向量丛模空间的经典用书. 第二部分有此学科最先进的结果. 各章的附录都有很重要又有趣的结果.主要内容包括半稳定丛的分解成稳定丛,稳定丛的陈数不等式(Bogomorov Inequality), Mumford 的几何不变量理论, 稳定向量丛模空间的制造, 曲面上向丛模空间的平滑性,不可约性(李骏的定理), K3曲面上向量丛模空间的性质. 这本书的语言有点形式化,有可能读的时候会失去几何直观.所以读者可以参考其他比较几何的书,比如Robert Friedman 的向量丛的书.------------------------- 困难度难趣味性*** 投资报酬率*****16 MK47 步枪Joyce, Gross & Huybrecht 的Calabi-Yau Manifolds and Related Geometries. 是最新的Mirror symmetry 的专题书. 讲Calabi Yau 流形的各种相关问题. 有Yau 解决Calabi 猜想的概述. 有Mirror 猜想和SYZ (Strominger& Yau& Zaslow) 猜想. 还有HyperKaeler 流形性质的讨论.这是二十一世纪的数学.想要了解Calabi Yau 流形的相关性质的人一定不想错过这本书.--------------------------------困难度难趣味性***** 投资报酬率*****17 机关枪(可以抢银行)Pandharipande, Sheldon Katz, Hori... 一群人合写的Mirror Symmetry . 除了Mirror conjecture 在五次三微流形(quintic three fold )的证明外, 还包括了Gopakuma Vafa 猜想, Homological Mirror Symmetry 猜想, 甚至Mirror Symmetry 的源头: 高能物理中的弦论和保角场论, 全都由专家执笔.. 从难到易..笔者也在修练中.----------------- 困难度极难(物理部分) 趣味性***** 投资报酬率*****18、原子弹(请在没有人类的地方阅读) Griffith 的Topics in Trascendental Geometry是霍奇结构(Hodge structure) 的一本经典书. 在1985年左右有一大票数学家想解决霍奇猜想(没错就是那个一百万问题).她们虽然没有解出来但对猜想有很深入的了解. 本书是她们工作的简述. 内容包括霍奇结构的变形,霍奇丛,Monodromy,混霍奇结构,Torelli定理, 霍奇结构的退化,是一本难读却很值得读的书. 如果想要解决霍奇猜想或者是其相关问题,就得阅读此书. 镜对称的一办理论其实就是卡拉比-丘流形的霍奇变形所制造的不变量.难度极难趣味性**********************投资报酬率****************************================================================== ========================另外还有几本书没有介绍..例如有关Hodge 理论有Claire Voisin 的Hodge Theory and Complex Algebraic Geometry 两册书, 是很新的Hodge 理论和cycle 理论的书,写的很详细.又比如Shrinivas 的Algebraic K theory, 论述了Quillen 连结K-theory 和Chow group 的工作. 这两样都是以后很有发展的方向. 一个刚解决的方向是Mori 的三维代数流形的Minimal Model Programm , 有非常多的专书.但因为这个问题刚刚被萧荫堂以及其他四个外国人解决(所有维度) 其投资报酬率已经是负的了.也就是说大家可以不用去管它因为没有问题可以做了.其实有很多书笔者并没有介绍,很大的原因是也没有读过所以无从介绍. 比如说KaiBehrend 最近写了Gromov Witten 专书, Tian Gang 写了Calabi 猜想相关的书.Dominic Joyce 也写了关于"special holonomy" 的书. Daniel Huybrecht写了一本关于曲面上层的Derived category的性质的书. 另外笔者认为, 所谓的Homotopical algebra(Andrew 和Quillen 在60 年代的专著书), Noncommutative Geometry(Allaine Cone 的工作也有专著), Flow 理论和Minimal Surface (比如Halmilton的Ricci flow, 或是Kaheler flow), 一种叫做Microlocal analysis (Kashiwara 有专著) 的理论, 无限维李代数的表示理论(Kacs Moody algebra), 数论相关的Motivic理论, Yang Mills 和Chern Simon 方程的理论, 都将对一百年内的袋鼠几何发生影响. 其中任何一个方向要学好都是非常花时间的事情,经通两项就已经难如登天了,希望各位袋鼠可以找到最喜欢的方向.。
[黎曼几何]比较定理的应用(直径估计、基本群)
![[黎曼几何]比较定理的应用(直径估计、基本群)](https://img.taocdn.com/s3/m/2e61ce55326c1eb91a37f111f18583d049640f78.png)
LECTURE40:APPLICATIONS OF THE VOLUME COMPARISONTHEOREM1.Volume Growth of Geodesic BallsLet(M,g)be a complete Riemannian manifold with Ric≥0.According to Bishop-Gromov volume comparison theorem,Vol(B r(p))≤Vol(B0r)=ωm r m,whereωm is the volume of unit ball in R m with equality holds if and only if(M,g)is isometric with(R m,g0).A natural question is:what is the lower bound of the volume growth?Of course this question is reasonable only for non-compact Riemannian manifolds.Theorem1.1(Calabi-Yau).Let(M,g)be a complete non-compact Riemannian man-ifold with Ric≥0.Then there exists a positive constant c depending only on p and m so thatVol(B r(p))≥crfor any r>2.Proof.(This proof is due to Gromov.)Since M is complete and non-compact,for any p∈M there exists a ray,i.e.a geodesicγ:[0,∞)→M withγ(0)=p such that dist(p,γ(t))=t for all t>0.(See PSet3problem4for more details.) For any t>32,using the Bishop-Gromov volume comparison theorem,we getVol(B t+1(γ(t))) Vol(B t−1(γ(t)))≤ωm(t+1)mωm(t−1)m=(t+1)m(t−1)m.On the other hand,by triangle inequality,B1(p)⊂B t+1(γ(t))\B t−1(γ(t)).It followsVol(B1(p)) Vol(B t−1(γ(t)))≤Vol(B t+1(γ(t))\B t−1(γ(t)))Vol(B t−1(γ(t)))≤(t+1)m−(t−1)m(t−1)m,i.e.Vol(B t−1(γ(t)))≥Vol(B1(p))(t−1)m(t+1)m−(t−1)m≥C(m)Vol(B1(p))t,wehre C(m)is the infimum of the function1t(t−1)m(t+1)m−(t−1)mon[32,∞),which is positive.Now the theorem follows from the factB r(p)⊃B r+12−1(γ(r+12)).12LECTURE40:APPLICATIONS OF THE VOLUME COMPARISON THEOREM2.Cheng’s Maximal Diameter TheoremAs a second application of volume comparison theorem,we will proveTheorem2.1(S.Y.Cheng).Let(M,g)be a complete Riemanniian manifold withRic≥(n−1)k for some k>0,and diam(M,g)=π√k ,then M is isometric to thestandard sphere of radius1√k.Proof.(This proof is due to Shiohama)For simplicity we may assume k=1.By Bishop-Gromov volume comparison theorem,for any p∈M,Vol(Bπ/2(p)) Vol(M)=Vol(Bπ/2(p))Vol(Bπ(p))≥Vol(B1π/2)Vol(B1π)=12.Now let p,q∈M so that dist(p,q)=π.The the above inequality impliesVol(Bπ/2(p))≥12Vol(M),Vol(Bπ/2(q))≥12Vol(M).Since Bπ/2(p)∩Bπ/2(q)=∅,we must haveVol(Bπ/2(p)) Vol(Bπ(p))=Vol(B1π/2)Vol(B1π)=12,Vol(Bπ/2(q))Vol(Bπ(q))=Vol(B1π/2)Vol(B1π)=12.According to Bishop-Gromov comparison theorem,Bπ/2(p)and Bπ/2(q)are both iso-metric to half sphere.It follows that M is isometric to S m.3.Fundamental Group and Milnor’s ConjectureLet’s start with some abstract definitions in algebra.Let G be a group.G is said to befinitely generated if there exists afinite subsetΓ={g1,···,g N}of G so that any element in G can be represented as group multiplications of elements inΓ.Note that if the group identity element e is inΓ,we can always remove it.Now let’sfix a setΓof generators of G.The growth function of G with respect toΓis defined to be the number of group elements that can be represented as a product of at most k generators,i.e.NΓG (k)=#{g∈G|∃l≤k and g i1,···,g il∈Γs.t.g=g i1···g il}.We say that G is of(at most)polynomial growth if NΓG (k)≤ck n for some constant cdepending only on G,Γ,and similarly G is of(at least)exponential growth if NΓG (k)≥ce k.Note that ifΓ is anotherfinite set of generators,then there exists integers c1,c2 so that any element ofΓcan be represented via at most c1elements ofΓ ,and any element ofΓ can be represented via at most c2elements ofΓ.It follows thatNΓG (k)≥NΓG(c1k),NΓG(k)≥NΓG(c2k).So the conception of polynomial/exponential growth is independent of the choice of the generating set.LECTURE40:APPLICATIONS OF THE VOLUME COMPARISON THEOREM3Coming back to Riemannian manifolds.If(M,g)is a compact Riemannian man-ifold,and M its universal covering endowed with pull-back metric.Then the funda-mental groupπ1(M)acts isometrically on M as the group of deck transformations.If M is compact,the following results are well-known:•π1(M)isfinitely generated.•(Gromov)If K≥0,then the set of generates can be choose to be no more than c(m)for some constant c depending only on m.A similar results holds for manifolds with K≥−k2and diam(M,g)≤D.(The proof uses Toporogov comparison theorem.)•(Milnor)If Ric≥0,then NΓ(k)≤ck m;if K<0,then NΓ(k)≥ce k.(The proof uses volume comparison theorem.See the following theorem for thefirst part.)For non-compact Riemannian manifolds,the fundamental group might be not finitely generated in general.However,we haveTheorem3.1(Milnor).Let M be a complete Riemannian manifold with Ric≥0 and let G⊂π1(M)be anyfinitely generated subgroup.Then there exists a constant c depending only on M and the chosefinite generating setΓof G so that NΓ(k)≤ck m. Proof.LetΓbe afinite set of generators of G.Fix a point˜p∈ M and letl=max{dist(˜p,g i˜p)|g i∈Γ}.Then by triangle inequality,for any g=g i1···g ik∈Γk⊂G,dist(˜p,g˜p)≤kl.One theother hand side,we can pickε=13min{dist(˜p,g˜p)|e=g∈G}>0so that the balls Bε(g˜p)are all disjoint for g∈G.It followsB kl+ε(˜p)⊃∪g∈Γk Bε(g˜p)and thusVol(B kl+ε(˜p))≥NΓGVol(Bε(p)). Applying the Bishop-Gromov’s volume comparison theorem,we getNΓG ≤Vol(B kl+ε(˜p))Vol(Bε(p))≤(kl+ε)mεm≤ck m.We end this course by stating the following major conjecture in this subject: Conjecture3.2(Milnor).Let M be a complete Riemannian manifold with Ric≥0, thenπ1(M)isfinitely generated.。
金兹堡朗道理论

Ginzburg–Landau theoryFrom Wikipedia, the free encyclopediaIn physics, Ginzburg–Landau theory, named after Vitaly Lazarevich Ginzburg and Lev Landau, is a mathematical physical theory used to describe superconductivity. In its initial form, it was postulated as a phenomenological model which could describe type-I superconductors without examining their microscopic properties. Later, a version of Ginzburg–Landau theory was derived from the Bardeen-Cooper-Schrieffer microscopic theory by Lev Gor'kov, thus showing that it also appears in some limit of microscopic theory and giving microscopic interpretation of all its parameters.Contents•1Introduction•2Simple interpretation•3Coherence length and penetration depth•4Fluctuations in the Ginzburg–Landau model•5Classification of superconductors based on Ginzburg–Landau theory•6Landau–Ginzburg theories in string theory•7See also•8References•8.1PapersIntroduction[edit]Based on Landau's previously-established theory of second-order phase transitions, Ginzburg and Landau argued that the free energy, F, of a superconductor near the superconducting transition can be expressed in terms ofa complex order parameter field, ψ, which is nonzero below a phase transition into a superconducting state and isrelated to the density of the superconducting component, although no direct interpretation of this parameter was given in the original paper. Assuming smallness of |ψ| and smallness of its gradients, the free energy has the form ofa field theory.where F n is the free energy in the normal phase, α and β in the initial argument were treated as phenomenologicalparameters, m is an effective mass, e is the charge of an electron, A is the magnetic vector potential, and is the magnetic field. By minimizing the free energy with respect to variations in the order parameter and the vector potential, one arrives at the Ginzburg–Landau equationswhere j denotes the dissipation-less electric current density and Re the real part. The first equation — which bears some similarities to the time-independent Schrödinger equation, but is principally different due to a nonlinear term —determines the order parameter, ψ. The second equation then provides the superconducting current.Simple interpretation[edit]Consider a homogeneous superconductor where there is no superconducting current and the equation for ψ simplifies to:This equation has a trivial solution: ψ = 0. This corresponds to the normal state of the superconductor, that is for temperatures above the superconducting transition temperature, T>T c.Below the superconducting transition temperature, the above equation is expected to have a non-trivial solution (that is ψ ≠ 0). Under this assumption the equation above can be rearranged into:When the right hand side of this equation is positive, there is a nonzero solution for ψ (remember that the magnitude of a complex number can be positive or zero). This can be achieved by assuming the following temperature dependence of α: α(T) = α0 (T - T c) with α0/ β > 0:•Above the superconducting transition temperature, T > T c, the expression α(T) / β is positive and the right hand side of the equation above is negative. The magnitude of a complex number must be a non-negative number, so only ψ = 0 solves the Ginzburg–Landau equation.•Below the superconducting transition temperature, T < T c, the right hand side of the equation above is positive and there is a non-trivial solution for ψ. Furthermorethat is ψ approaches zero as T gets closer to T c from below. Such a behaviour is typical for a second order phase transition.In Ginzburg–Landau theory the electrons that contribute to superconductivity were proposed to forma superfluid.[1] In this interpretation, |ψ|2 indicates the fraction of electrons that have condensed into a superfluid.[1] Coherence length and penetration depth[edit]The Ginzburg–Landau equations predicted two new characteristic lengths in a superconductor which wastermed coherence length, ξ. For T > T c (normal phase), it is given bywhile for T < T c (superconducting phase), where it is more relevant, it is given byIt sets the exponential law according to which small perturbations of density of superconducting electrons recover their equilibrium value ψ0. Thus this theory characterized all superconductors by two length scales. The second one is the penetration depth, λ. It was previously introduced by the London brothers in their London theory. Expressed in terms of the parameters of Ginzburg-Landau model it iswhere ψ0 is the equilibrium value of the order parameter in the absence of an electromagnetic field. The penetration depth sets the exponential law according to which an external magnetic field decays inside the superconductor. The original idea on the parameter "k" belongs to Landau. The ratio κ = λ/ξ is presently known asthe Ginzburg–Landau parameter. It has been proposed by Landau that Type I superconductors are those with 0 < κ< 1/√2, and Type II superconductors those with κ> 1/√2.The exponential decay of the magnetic field is equivalent with the Higgs mechanism in high-energy physics. Fluctuations in the Ginzburg–Landau model[edit]Taking into account fluctuations. For Type II superconductors, the phase transition from the normal state is of second order, as demonstrated by Dasgupta and Halperin. While for Type I superconductors it is of first order as demonstrated by Halperin, Lubensky and Ma.Classification of superconductors based on Ginzburg–Landau theory[edit]In the original paper Ginzburg and Landau observed the existence of two types of superconductors depending on the energy of the interface between the normal and superconducting states.The Meissner state breaks down when the applied magnetic field is too large. Superconductors can be divided into two classes according to how this breakdown occurs. In Type I superconductors, superconductivity is abruptly destroyed when the strength of the applied field rises above a critical value H c. Depending on the geometry of the sample, one may obtain an intermediate state[2] consisting of a baroque pattern[3] of regions of normal material carrying a magnetic field mixed with regions of superconducting material containing no field. In Type II superconductors, raising the applied field past a critical value H c1 leads to a mixed state (also known as the vortex state) in which an increasing amount of magnetic flux penetrates the material, but there remains no resistance to the flow of electric current as long as the current is not too large. At a second critical field strength H c2, superconductivity is destroyed. The mixed state is actually caused by vortices in the electronic superfluid, sometimes called fluxons because the flux carried by these vortices is quantized. Most pure elemental superconductors, except niobium and carbon nanotubes, are Type I, while almost all impure and compound superconductors are Type II.The most important finding from Ginzburg–Landau theory was made by Alexei Abrikosov in 1957. He used Ginzburg–Landau theory to explain experiments on superconducting alloys and thin films. He found that in a type-II superconductor in a high magnetic field, the field penetrates in a triangular lattice of quantized tubes offlux vortices.[citation needed]Landau–Ginzburg theories in string theory[edit]In particle physics, any quantum field theory with a unique classical vacuum state and a potential energy witha degenerate critical point is called a Landau–Ginzburg theory. The generalization to N=(2,2) supersymmetric theories in 2 spacetime dimensions was proposed by Cumrun Vafa and Nicholas Warner in the November 1988 article Catastrophes and the Classification of Conformal Theories, in this generalization one imposes thatthe superpotential possess a degenerate critical point. The same month, together with Brian Greene they argued that these theories are related by a renormalization group flow to sigma models on Calabi–Yau manifolds in thepaper Calabi–Yau Manifolds and Renormalization Group Flows. In his 1993 paper Phases of N=2 theories intwo-dimensions, Edward Witten argued that Landau–Ginzburg theories and sigma models on Calabi–Yau manifolds are different phases of the same theory. A construction of such a duality was given by relating the Gromov-Witten theory of Calabi-Yau orbifolds to FJRW theory an analogous Landau-Ginzburg "FJRW" theory in The Witten Equation, Mirror Symmetry and Quantum Singularity Theory. Witten's sigma models were later used to describe the low energy dynamics of 4-dimensional gauge theories with monopoles as well as brane constructions. Gaiotto, Gukov & Seiberg (2013)See also[edit]•Domain wall (magnetism)•Flux pinning•Gross–Pitaevskii equation•Husimi Q representation•Landau theory•Magnetic domain•Magnetic flux quantum•Reaction–diffusion systems•Quantum vortex•Topological defectReferences[edit]1.^ Jump up to:a b Ginzburg VL (July 2004). "On superconductivity and superfluidity (what I have and havenot managed to do), as well as on the 'physical minimum' at the beginning of the 21 st century". Chemphyschem.5 (7): 930–945. doi:10.1002/cphc.200400182. PMID15298379.2.Jump up^ Lev D. Landau; Evgeny M. Lifschitz (1984). Electrodynamics of Continuous Media. Course ofTheoretical Physics8. Oxford: Butterworth-Heinemann. ISBN0-7506-2634-8.3.Jump up^ David J. E. Callaway (1990). "On the remarkable structure of the superconductingintermediate state". Nuclear Physics B344 (3): 627–645. Bibcode:1990NuPhB.344..627C.doi:10.1016/0550-3213(90)90672-Z.Papers[edit]•V.L. Ginzburg and L.D. Landau, Zh. Eksp. Teor. Fiz.20, 1064 (1950). English translation in: L. D. Landau, Collected papers (Oxford: Pergamon Press, 1965) p. 546• A.A. Abrikosov, Zh. Eksp. Teor. Fiz.32, 1442 (1957) (English translation: Sov. Phys. JETP5 1174 (1957)].) Abrikosov's original paper on vortex structure of Type-II superconductors derived as a solution of G–L equations for κ > 1/√2•L.P. Gor'kov, Sov. Phys. JETP36, 1364 (1959)• A.A. Abrikosov's 2003 Nobel lecture: pdf file or video•V.L. Ginzburg's 2003 Nobel Lecture: pdf file or video•Gaiotto, David; Gukov, Sergei; Seiberg, Nathan (2013), "Surface Defects and Resolvents" (PDF), Journal of High Energy Physics。
Discrete Torsion in Singular G_2-Manifolds and Real LG

¨ R. ROIBAN, C. ROMELSBERGER and J. WALCHER
209
description is in terms of Landau-Ginzburg theory [30–34]. For Y at hand, the relevant LG model is given by the superpotential
roemel@
Abstract We investigate strings at singularities of G2 -holonomy manifolds which arise in Z2 orbifolds of Calabi-Yau spaces times a circle. The singularities locally look like R4 /Z2 fibered over a SLAG, and can globally be embedded in CICYs in weighted projective spaces. The local model depends on the choice of a discrete torsion in the fibration, and the global model on an anti-holomorphic involution of the CalabiYau hypersurface. We determine how these choices are related to each other by computing a Wilson surface detecting discrete torsion. We then follow the same orbifolds to the non-geometric Landau-Ginzburg region of moduli space. We argue that the symmetry-breaking twisted sectors are effectively captured by real Landau-Ginzburg potentials. In particular, we find agreement in the low-energy spectra of strings computed from geometry and Gepner-model CFT. Along the way, we construct the full modular data of orbifolds of N = 2 minimal models by the mirror automorphism, and give a real-LG interpretation of their modular invariants. Some of the models provide examples of the mirror-symmetry phenomenon for G2 holonomy. e-print archive: /abs/hep-th/0203272
食管鳞癌组织中VEGFR-2基因扩增情况观察及与患者预后关系分析

食管鳞癌组织中VEGFR -2基因扩增情况观察及与患者预后关系分析吐尔洪·托合提1,阿丽米热·库尔班2,卡吾力·居买1,居来提·艾尼瓦尔1,张海平1,伊地力斯·阿吾提11 新疆医科大学第一附属医院,乌鲁木齐830054;2 新疆医科大学基础医学院摘要:目的 观察食管鳞癌组织中血管内皮生长因子受体-2(VEGFR -2)基因扩增情况,并分析VEGFR -2基因扩增与食管鳞癌患者预后的关系。
方法 食管鳞癌患者120例,术中留取食管癌组织,采用荧光原位杂交(FISH )法观察食管鳞癌组织中VEGFR -2基因扩增情况,并分析VEGFR -2基因扩增与食管鳞癌患者临床病理参数的关系。
根据有无VEGFR -2基因扩增,将120例食管鳞癌患者分为阳性组(VEGFR -2基因扩增阳性)和阴性组(VEGFR -2基因扩增阴性),采用Kaplan -Meier 生存分析法及多因素COX 回归模型分析VEGFR -2基因扩增与食管鳞癌患者预后的关系。
结果 120例食管鳞癌患者中,49例患者存在VEGFR -2基因扩增,VEGFR -2基因扩增率为40.8%。
VEGFR -2基因扩增与食管鳞癌患者肿瘤分化程度、有无淋巴结转移、有无脉管浸润有关(P 均<0.05)。
阴性组的食管鳞癌患者生存时间为16(11.15~20.84)个月,阳性组为13(8.27~17.72)个月,两组相比,P <0.05。
多因素COX 回归分析结果显示,VEGFR -2基因扩增是食管鳞癌患者不良预后的独立危险因素(HR=0.562,95%CI :0.374~0.844,P =0.006)。
结论 部分食管鳞癌患者存在VEGFR -2基因扩增。
VEGFR -2基因扩增与食管鳞癌肿瘤分化程度、有无淋巴结转移、有无脉管浸润有关,是食管鳞癌患者不良预后的独立危险因素。
关键词:血管内皮生长因子受体-2基因;基因扩增;食管癌;食管鳞状细胞癌doi :10.3969/j.issn.1002-266X.2023.09.008中图分类号:R 文献标志码:A 文章编号:1002-266X (2023)09-0031-05VEGFR -2 gene amplification in esophageal squamous cell carcinoma and its relationship with prognosis Turgon Tohti 1, Alimire Kurban , Kawul Juma , Jurat Anwar , ZHANG Haiping , Edris Awut 1 The First Affiliated Hospital of Xinjiang Medical University , Urumqi 830054, ChinaAbstract : Objective To observe the amplification of vascular endothelial growth factor receptor -2 (VEGFR -2)gene in esophageal squamous cell carcinoma , and to analyze the relationship between the amplification of VEGFR -2 gene and the prognosis of esophageal squamous cell carcinoma patients. Methods In 120 patients with esophageal squamous cell carcinoma , esophageal cancer tissues were taken during operation. The amplification of VEGFR -2 gene in esophageal squamous cell carcinoma tissue was observed by fluorescence in situ hybridization (FISH ), and the relationships between the amplification of VEGFR -2 gene and the clinicopathologic parameters of esophageal squamous cell carcinoma patients were analyzed. According to the presence or absence of VEGFR -2 gene amplification , 120 patients with esophageal squa‐mous cell carcinoma were divided into the positive group (VEGFR -2 gene amplification positive ) and negative group(VEGFR -2 gene amplification negative ). The relationship between VEGFR -2 gene amplification and prognosis of patients with esophageal squamous cell carcinoma was analyzed by Kaplan -Meier survival analysis and multivariate COX regres‐sion model. Results Among 120 patients with esophageal squamous cell carcinoma , 49 patients had VEGFR -2 gene am‐plification , and the amplification rate of VEGFR -2 gene was 40.8%. The amplification of VEGFR -2 gene was related to the degree of tumor differentiation , lymph node metastasis and vascular invasion in patients with esophageal squamous cell carcinoma (all P<0.05). The survival time of esophageal squamous cell carcinoma patients in the negative group was 16 (11.15–20.84) months , and that in the positive group was 13 (8.27–17.72) months , with statistically significant dif‐ference (P<0.05). Multivariate COX regression analysis showed that VEGFR -2 gene amplification was an independent基金项目:国家自然科学基金资助项目(81960498)。
Heat-kernel Coefficients and Spectra of the Vector Laplacians on Spherical Domains with Con

ds K (s ) , s
(1.1)
where δ 2 is an ultraviolet cut–off. The important fact is that the conical singularities change the structure of the ultraviolet divergences Wdiv of the action (1.1), which in four dimensions has the form 1 Wdiv = − 32π 2 1 1 A0 + 2 A1 − ln δ 2 A2 4 2δ δ , (1.2)
Quantum field theory on spaces Mβ with conical singularities is a research subject which recently many publications have been devoted to. Conical singularities are a set Σ of points near which Mβ have the structure Cβ × Σ where Cβ is a cone with an angle β . The well–known example of such situation in physics is a space–time around an idealized infinitely thin cosmic string [1]. Spaces like Mβ appear in the black hole thermodynamics as well, and for this reason they have been carefully investigated in the last years. In the Gibbons–Hawking formulation [2] the free energy of a black hole is defined in terms of the gravitational action on the Euclidean black hole instanton. The regularity condition of such instantons near the Euclidean horizon picks up a period β of the imaginary time which is equal to the −1 inverse Hawking temperature TH . On the other hand, the mass M of a black hole is uniquely related to its Hawking temperature TH and, hence the Hamiltonian describing quantum excitations on the black hole background depends on the temperature as well [3]. However, the statistical–mechanical definition of the entropy and other quantities usually requires calculation of the partial derivatives of the free energy on the temperature. For the black holes it implies that one has to deal with the temperatures which are different from the Hawking value and independent on the mass M . In this case the black hole instanton is a space like Mβ , and has a conical singularity on the Euclidean horizon Σ. The corresponding approach to the black hole thermodynamics is called the off–shell approach [4, 5], because singular spaces do not obey the vacuum Einstein equations. In the Einstein gravity the off–shell derivation gives the correct Bekenstein–Hawking value for the black hole entropy [6]. The off–shell method can be also generalized to the higher–derivative gravity theories [7] and it enables one to get the black hole entropy which agrees with the other methods. The search for the statistical–mechanical explanation of the black hole entropy [8] has given rise to the off–shell methods in the thermodynamics of quantum black holes (see for review [5]). The methods, which use manifolds with conical singularities, were formulated and investigated for the static [10]–[12],[5] and for the rotating black holes [13, 14]. On curved backgrounds the one–loop effective action W is defined in terms of the trace K (s) of the heat kernel for the Laplace operator △ of the corresponding field, by equation 1 1 W = − log det △ = − 2 2
金兹堡朗道理论

Ginzburg–Landau theoryFrom Wikipedia, the free encyclopediaIn physics, Ginzburg–Landau theory, named after Vitaly Lazarevich Ginzburg and Lev Landau, is a mathematical physical theory used to describe superconductivity. In its initial form, it was postulated as a phenomenological model which could describe type-I superconductors without examining their microscopic properties. Later, a version of Ginzburg–Landau theory was derived from the Bardeen-Cooper-Schrieffer microscopic theory by Lev Gor'kov, thus showing that it also appears in some limit of microscopic theory and giving microscopic interpretation of all its parameters.Contents•1Introduction•2Simple interpretation•3Coherence length and penetration depth•4Fluctuations in the Ginzburg–Landau model•5Classification of superconductors based on Ginzburg–Landau theory•6Landau–Ginzburg theories in string theory•7See also•8References•8.1PapersIntroduction[edit]Based on Landau's previously-established theory of second-order phase transitions, Ginzburg and Landau argued that the free energy, F, of a superconductor near the superconducting transition can be expressed in terms ofa complex order parameter field, ψ, which is nonzero below a phase transition into a superconducting state and isrelated to the density of the superconducting component, although no direct interpretation of this parameter was given in the original paper. Assuming smallness of |ψ| and smallness of its gradients, the free energy has the form ofa field theory.where F n is the free energy in the normal phase, α and β in the initial argument were treated as phenomenologicalparameters, m is an effective mass, e is the charge of an electron, A is the magnetic vector potential, and is the magnetic field. By minimizing the free energy with respect to variations in the order parameter and the vector potential, one arrives at the Ginzburg–Landau equationswhere j denotes the dissipation-less electric current density and Re the real part. The first equation — which bears some similarities to the time-independent Schrödinger equation, but is principally different due to a nonlinear term —determines the order parameter, ψ. The second equation then provides the superconducting current.Simple interpretation[edit]Consider a homogeneous superconductor where there is no superconducting current and the equation for ψ simplifies to:This equation has a trivial solution: ψ = 0. This corresponds to the normal state of the superconductor, that is for temperatures above the superconducting transition temperature, T>T c.Below the superconducting transition temperature, the above equation is expected to have a non-trivial solution (that is ψ ≠ 0). Under this assumption the equation above can be rearranged into:When the right hand side of this equation is positive, there is a nonzero solution for ψ (remember that the magnitude of a complex number can be positive or zero). This can be achieved by assuming the following temperature dependence of α: α(T) = α0 (T - T c) with α0/ β > 0:•Above the superconducting transition temperature, T > T c, the expression α(T) / β is positive and the right hand side of the equation above is negative. The magnitude of a complex number must be a non-negative number, so only ψ = 0 solves the Ginzburg–Landau equation.•Below the superconducting transition temperature, T < T c, the right hand side of the equation above is positive and there is a non-trivial solution for ψ. Furthermorethat is ψ approaches zero as T gets closer to T c from below. Such a behaviour is typical for a second order phase transition.In Ginzburg–Landau theory the electrons that contribute to superconductivity were proposed to forma superfluid.[1] In this interpretation, |ψ|2 indicates the fraction of electrons that have condensed into a superfluid.[1] Coherence length and penetration depth[edit]The Ginzburg–Landau equations predicted two new characteristic lengths in a superconductor which wastermed coherence length, ξ. For T > T c (normal phase), it is given bywhile for T < T c (superconducting phase), where it is more relevant, it is given byIt sets the exponential law according to which small perturbations of density of superconducting electrons recover their equilibrium value ψ0. Thus this theory characterized all superconductors by two length scales. The second one is the penetration depth, λ. It was previously introduced by the London brothers in their London theory. Expressed in terms of the parameters of Ginzburg-Landau model it iswhere ψ0 is the equilibrium value of the order parameter in the absence of an electromagnetic field. The penetration depth sets the exponential law according to which an external magnetic field decays inside the superconductor. The original idea on the parameter "k" belongs to Landau. The ratio κ = λ/ξ is presently known asthe Ginzburg–Landau parameter. It has been proposed by Landau that Type I superconductors are those with 0 < κ< 1/√2, and Type II superconductors those with κ> 1/√2.The exponential decay of the magnetic field is equivalent with the Higgs mechanism in high-energy physics. Fluctuations in the Ginzburg–Landau model[edit]Taking into account fluctuations. For Type II superconductors, the phase transition from the normal state is of second order, as demonstrated by Dasgupta and Halperin. While for Type I superconductors it is of first order as demonstrated by Halperin, Lubensky and Ma.Classification of superconductors based on Ginzburg–Landau theory[edit]In the original paper Ginzburg and Landau observed the existence of two types of superconductors depending on the energy of the interface between the normal and superconducting states.The Meissner state breaks down when the applied magnetic field is too large. Superconductors can be divided into two classes according to how this breakdown occurs. In Type I superconductors, superconductivity is abruptly destroyed when the strength of the applied field rises above a critical value H c. Depending on the geometry of the sample, one may obtain an intermediate state[2] consisting of a baroque pattern[3] of regions of normal material carrying a magnetic field mixed with regions of superconducting material containing no field. In Type II superconductors, raising the applied field past a critical value H c1 leads to a mixed state (also known as the vortex state) in which an increasing amount of magnetic flux penetrates the material, but there remains no resistance to the flow of electric current as long as the current is not too large. At a second critical field strength H c2, superconductivity is destroyed. The mixed state is actually caused by vortices in the electronic superfluid, sometimes called fluxons because the flux carried by these vortices is quantized. Most pure elemental superconductors, except niobium and carbon nanotubes, are Type I, while almost all impure and compound superconductors are Type II.The most important finding from Ginzburg–Landau theory was made by Alexei Abrikosov in 1957. He used Ginzburg–Landau theory to explain experiments on superconducting alloys and thin films. He found that in a type-II superconductor in a high magnetic field, the field penetrates in a triangular lattice of quantized tubes offlux vortices.[citation needed]Landau–Ginzburg theories in string theory[edit]In particle physics, any quantum field theory with a unique classical vacuum state and a potential energy witha degenerate critical point is called a Landau–Ginzburg theory. The generalization to N=(2,2) supersymmetric theories in 2 spacetime dimensions was proposed by Cumrun Vafa and Nicholas Warner in the November 1988 article Catastrophes and the Classification of Conformal Theories, in this generalization one imposes thatthe superpotential possess a degenerate critical point. The same month, together with Brian Greene they argued that these theories are related by a renormalization group flow to sigma models on Calabi–Yau manifolds in thepaper Calabi–Yau Manifolds and Renormalization Group Flows. In his 1993 paper Phases of N=2 theories intwo-dimensions, Edward Witten argued that Landau–Ginzburg theories and sigma models on Calabi–Yau manifolds are different phases of the same theory. A construction of such a duality was given by relating the Gromov-Witten theory of Calabi-Yau orbifolds to FJRW theory an analogous Landau-Ginzburg "FJRW" theory in The Witten Equation, Mirror Symmetry and Quantum Singularity Theory. Witten's sigma models were later used to describe the low energy dynamics of 4-dimensional gauge theories with monopoles as well as brane constructions. Gaiotto, Gukov & Seiberg (2013)See also[edit]•Domain wall (magnetism)•Flux pinning•Gross–Pitaevskii equation•Husimi Q representation•Landau theory•Magnetic domain•Magnetic flux quantum•Reaction–diffusion systems•Quantum vortex•Topological defectReferences[edit]1.^ Jump up to:a b Ginzburg VL (July 2004). "On superconductivity and superfluidity (what I have and havenot managed to do), as well as on the 'physical minimum' at the beginning of the 21 st century". Chemphyschem.5 (7): 930–945. doi:10.1002/cphc.200400182. PMID15298379.2.Jump up^ Lev D. Landau; Evgeny M. Lifschitz (1984). Electrodynamics of Continuous Media. Course ofTheoretical Physics8. Oxford: Butterworth-Heinemann. ISBN0-7506-2634-8.3.Jump up^ David J. E. Callaway (1990). "On the remarkable structure of the superconductingintermediate state". Nuclear Physics B344 (3): 627–645. Bibcode:1990NuPhB.344..627C.doi:10.1016/0550-3213(90)90672-Z.Papers[edit]•V.L. Ginzburg and L.D. Landau, Zh. Eksp. Teor. Fiz.20, 1064 (1950). English translation in: L. D. Landau, Collected papers (Oxford: Pergamon Press, 1965) p. 546• A.A. Abrikosov, Zh. Eksp. Teor. Fiz.32, 1442 (1957) (English translation: Sov. Phys. JETP5 1174 (1957)].) Abrikosov's original paper on vortex structure of Type-II superconductors derived as a solution of G–L equations for κ > 1/√2•L.P. Gor'kov, Sov. Phys. JETP36, 1364 (1959)• A.A. Abrikosov's 2003 Nobel lecture: pdf file or video•V.L. Ginzburg's 2003 Nobel Lecture: pdf file or video•Gaiotto, David; Gukov, Sergei; Seiberg, Nathan (2013), "Surface Defects and Resolvents" (PDF), Journal of High Energy Physics。
Shut up and calculate

The foundation of my argument is the assumption that there exists an external physical reality independent of us humans. This is not too controversial: I would guess that the majority of physicists favour this long-standing idea, though it is still debated. Metaphysical solipsists reject it flat out, and supporters of the so-called Copenhagen interpretation of quantum mechanics may reject it on the grounds that there is no reality without observation (New Scientist, 23 June, p 30). Assuming an external reality exists, physics theories aim to describe how it works. Our most successful theories, such as general relativity and quantum mechanics, describe only parts of this reality: gravity, for instance, or the behaviour of subatomic particles. In contrast, the holy grail of theoretical physics is a theory of everything — a complete description of reality.
longikaurin a的结构式

longikaurin a的结构式全文共四篇示例,供读者参考第一篇示例:长驱漫漫山河远,千锤百炼始成钢。
在有机化学领域,无数科学家们不断地探索新的化合物,寻找新的治疗方法和药物。
今天,我们要介绍的是一种名为longikaurin a的有机化合物,它具有独特的结构和潜在的药用价值。
Longikaurin a是一种从植物中提取的次生代谢产物,具有复杂的结构,属于类醌类化合物。
它的结构式为C25H24O8,由一个螺环和一个二氧杂环构成。
长链结构和多环结构使得longikaurin a在天然界中比较罕见,但却具有许多引人注目的生物活性。
研究表明,longikaurin a具有抗炎、抗菌、抗肿瘤等多种药理活性。
其抗炎作用主要表现在抑制炎症介质的释放和调控炎症反应过程。
对于许多炎症性疾病,如风湿性关节炎、炎症性肠病等,longikaurin a的抗炎活性可能具有重要意义。
长期以来人们一直致力于寻找新的抗菌药物,longikaurin a的抗菌活性也受到了广泛关注。
研究发现,它对多种病原微生物具有良好的抑制效果,包括耐药菌株。
这为开发新型抗菌药物提供了新的思路。
longikaurin a还表现出一定的抗肿瘤活性。
研究显示,它可以通过多种途径抑制肿瘤细胞的增殖和转移,诱导肿瘤细胞凋亡。
这使得longikaurin a在肿瘤治疗领域具有重要的潜在应用价值。
虽然目前其具体的抗肿瘤机制还需进一步研究,但长期的实验表明其在临床前研究中具有广阔的前景。
第二篇示例:长显剑藻素A(Longikaurin A)是一种从藻类中提取的天然产物,被广泛应用于药物研究和药理学领域。
这种化合物具有独特的结构,具有抗炎、抗肿瘤、抗菌等多种生物活性。
在本文中,将详细介绍长显剑藻素A的结构式以及其药理学特性。
长显剑藻素A的化学结构如下图所示:在上图中,我们可以看到长显剑藻素A的分子式为C30H33Cl2N3O5,分子量为594.51 g/mol。
小而密低密度脂蛋白与心血管疾病相关性的研究进展

㊃综述㊃小而密低密度脂蛋白与心血管疾病相关性的研究进展木那瓦尔㊃克热木㊀迪拉热㊃阿迪㊀魏娴㊀艾比班木㊃艾则孜㊀马依彤830011乌鲁木齐,新疆医科大学第一附属医院心脏中心冠心病科通信作者:马依彤,电子信箱:myt-xj@DOI:10.3969/j.issn.1007-5410.2023.06.016㊀㊀ʌ摘要ɔ㊀小而密低密度脂蛋白(sdLDL)为低密度脂蛋白亚型,是一种运输胆固醇进入外周组织细胞的脂蛋白颗粒㊂鉴于sdLDL较其他血脂成分有更强的导致动脉粥样硬化的能力,其被列为心血管疾病的新兴危险因素,已成为降血脂和抗动脉粥样硬化的药物研发新靶点㊂本文就sdLDL致动脉粥样硬化的分子机制及其与心血管疾病相关性进行系统综述,展望sdLDL作为降脂治疗靶标的潜能㊂ʌ关键词ɔ㊀小而密低密度脂蛋白;㊀心血管疾病;㊀药物干预靶点基金项目:国家自然科学基金(91957208㊁82260176);中央引导地方科技发展专项资金(ZYYD2022A01)Research progress on the association between small dense low density lipoprotein and cardiovasculardisease㊀Munawaer Keremu,Dilare Adi,Wei Xian,Aibibanmu Aizezi,Ma YitongDepartment of Coronary Heart Disease,Heart Center,The First Affiliated Hospital of Xinjiang MedicalUniversity,Urumqi830011,ChinaCorresponding author:Ma Yitong,Email:myt-xj@ʌAbstractɔ㊀Small dense low-density lipoprotein(sdLDL)is a subtype of low-density lipoprotein that transports cholesterol into peripheral tissue cells.Due to its stronger ability to induce atherosclerosis compared to other lipid components,sdLDL has been recognized as an emerging risk factor for cardiovasculardisease and has become a new target for lipid-lowering and anti-atherosclerosis drug development.Thisarticle provides a systematic review of the molecular mechanisms underlying sdLDL-induced atherosclerosisand its association with cardiovascular disease,and discusses the potential of sdLDL as a target for lipid-lowering therapy.ʌKey wordsɔ㊀Small dense low density lipoprotein;㊀Cardiovascular disease;㊀Drug intervention targetFund program:National Natural Science Foundation of China(91957208,82260176);Special FundProject of Central Government for Guiding Local Science and Technology Development(ZYYD2022A01)㊀㊀心血管疾病(cardiovascular disease,CVD)已成为严重危害人类健康的慢性非传染性疾病,是我国居民死亡和疾病负担的首要原因[1]㊂以低密度脂蛋白(low density lipoprotein, LDL)为 元凶 的动脉粥样硬化(atherosclerosis,AS)是CVD 的病理基础,尽管以他汀类药物为基石㊁多种降脂药物,如依折麦布及前蛋白转化酶枯草溶菌素9(proprotein convertase subtilisin/kexin type9,PCSK9)抑制剂为辅助的强化降脂治疗策略已广泛应用于CVD的二级预防,但仅使心血管事件的相对风险降低15%~25%[2],表明仍存在残余风险未得到有效控制㊂因此,寻求有效风险预测因子及干预靶点已成为防治CVD的根本㊂大量研究表明AS的发生不仅与LDL的数量有关,还与其异质性更相关,1983年,Fisher等[3]首次提出LDL具有异质性,可分为大而轻型和小而密型㊂小而密低密度脂蛋白(small dense LDL,sdLDL)为LDL的亚型,因颗粒小㊁低LDL 受体亲和力及易被修饰等特性,于2002年首次被国家胆固醇教育计划成人治疗组认为是LDL颗粒中更易导致AS发生的主要 坏胆固醇 ,是CVD重要危险因素,亦是关键预测因子[4]㊂此外,相关研究表明sdLDL在代谢综合征[5]㊁2型糖尿病[6]㊁肥胖[7]㊁高脂血症[8]㊁脂肪肝[9]等心血管相关代谢性疾病的发生发展中发挥着重要作用㊂本文将在阐述sdLDL的生成㊁致AS的发病机制及其与CVD相关性的基础上,探讨sdLDL作为新型降血脂和抗动脉粥样硬化的药物干预靶点的潜能㊂1㊀sdLDL概述1.1㊀sdLDL定义sdLDL作为LDL的亚型,主要是通过分离法而获得㊂Krauss等[10]首次应用超速离心法根据LDL密度将其分为四种亚型:LDLⅠ型(1.025~1.034g/ml)㊁LDLⅡ型(1.035~1.044g/ml)㊁LDLⅢ型(1.045~1.060g/ml)以及LDLⅣ型,并把密度ȡ1.045g/ml的亚型(LDLⅢ型和LDLⅣ型)称为sdLDL;随后Austin等[11]利用梯度凝胶电泳法,根据LDL颗粒大小和形状分为四种亚型:LDLⅠ型(大LDL, 26.0~28.5nm)㊁LDLⅡ型(中间LDL,25.5~26.4nm)㊁LDL ⅢA型和LDLⅢB型(小LDL,24.2~25.5nm)以及LDLⅣA 型和LDLⅣB型(极小的LDL,22.0~24.1nm),并根据粒径峰值分成两种表型:A型(LDLⅠ型和LDLⅡ型)>25.5nm 及B型(LDLⅢ型和LDLⅣ型)ɤ25.5nm,B型被称为sdLDL㊂此外,Hoefner等[12]应用Lipoprint TM系统,通过聚丙烯酰胺凝胶电泳法,根据电荷和粒径将LDL分为7个亚组分,其中组分3~7被称为sdLDL㊂因此,sdLDL被定义为粒径ɤ25.5nm㊁密度为1.045~1.060g/ml的脂蛋白颗粒㊂1.2㊀sdLDL检测方法目前已有多种实验室方法用于检测sdLDL水平㊂其中,超速离心法㊁梯度凝胶电泳法的操作复杂㊁耗时久,而其他如核磁共振光谱法㊁化学沉淀法㊁离子迁移率分析㊁高效液相色谱法等缺乏统一的操作标准,均不适合临床常规检测[13]㊂Hirano等[14]首次应用均相酶法,即通过表面活性剂和鞘磷脂酶去除sdLDL以外的脂蛋白进而测定其水平,该法快速㊁简便,已广泛用于sdLDL的大规模试验分析㊂sdLDL水平还可通过其他脂蛋白进行估计㊂Srisawasdi 等[15]通过LDL㊁非高密度脂蛋白(non high density lipoprotein,nonHDL)㊁直接测量的LDL(direct LDL,dLDL)建立了sdLDL公式:sdLDL(mg/dl)=0.580ˑnonHDL+0.407ˑdLDL-0.719ˑcLDL-12.05sdLDL(mmol/L)=0.580ˑnonHDL+0.407ˑdLDL-0.719ˑcLDL-0.312注:cLDL(采用Friedewald公式计算出的LDL)=nonHDL-TGː5,nonHDL=TC-HDL(TC为总胆固醇),TG为三酰甘油此外,Sampson[16]等也提出了新的sdLDL估算公式: ElbLDL=1.43ˑcLDL-[0.14ˑ(ln(TG)ˑcLDL]-8.99; EsdLDL=cLDL-ElbLDL注:cLDL(采用Sampson公式计算出的LDL);ElbLDL (使用公式估算出的大而轻型LDL)虽然已有几种方法用于检测sdLDL,但尚未制定统一的检测标准,因此需要更多研究为sdLDL分析提供最简便且准确的试验室方法㊂1.3㊀sdLDL的生成针对sdLDL的来源,Berneis等[17]提出两种与肝源性三酰甘油(triglyceride,TG)利用率有关的生成途径㊂肝脏基于合成的TG含量分为两种极低密度脂蛋白(very low density lipoprotein,VLDL)和中间密度脂蛋白(intermediate density lipoprotein,IDL),其中VLDL1和IDL1为富含TG,而VLDL2和IDL2为缺乏TG㊂当肝脏合成的TG增多时,肝脏直接分泌VLDL1和VLDL2,而当合成的TG减少时,则分泌VLDL1和IDL2,其中VLDL1在脂蛋白脂肪酶(lipoprotein lipase, LPL)和肝脂肪酶(hepatic lipase,HL)的作用下分解为LDL Ⅲ和LDLⅣ,而VLDL2在LPL的作用下分解为IDL1,富含TG的IDL1被LPL和HL分解为LDLⅡ,IDL2则被LPL分解为LDLⅠ㊂此外,VLDL和LDL之间存在着由胆固醇脂质转运蛋白(cholesteryl ester transfer protein,CETP)介导的脂质交换过程,在CETP的催化下,LDL内的总胆固醇转移至VLDL,而VLDL内的TG转移至LDL㊂富含TG的LDL是HL的底物,被HL分解后去除TG,转变为颗粒更小的LDL 亚型 sdLDL[18](图1)㊂因此,LDLⅢ和LDLⅣ为LDL颗粒中被HL分解生成sdLDL的主要亚型㊂TG:三酰甘油;VLDL:极低密度脂蛋白;LPL:脂蛋白脂肪酶;HL:肝脂肪酶;LDL:低密度脂蛋白;IDL:中间密度脂蛋白;sdLDL:小而密低密度脂蛋白;CETP:胆固醇脂质转运蛋白图1㊀sdLDL来源于两种肝源性三酰甘油可用性的途径1.4㊀sdLDL与遗传变异研究发现血液循环中的sdLDL水平受遗传因素的影响㊂Hoogeveen等[19]的一项全基因组关联研究结果显示,14个基因(或基因簇)的127个单核苷酸多态性(single nucleotide polymorphisms,SNPs)与血浆sdLDL水平存在显著相关性(P<5ˑ10-8)(表1)㊂其中,PCSK7基因的rs508487位点不同基因型的sdLDL水平存在显著差异,TT基因型携带者的sdLDL水平显著高于CT和CC基因型携带者(P<0.0001);此外,对PCSK7基因进行全外显子组测序后发现,与野生型相比,携带非同义突变者的血浆sdLDL水平显著升高(ʈ7.5 mg/dl,P=0.012)㊂Tsuzaki等[20]对时钟基因(circadian locomotor output cycles protein kaput,Clock)的3111T/C位点进行基因型分型后发现,Clock基因的3111T/C位点与血浆sdLDL水平存在相关性,T/T纯合子基因型携带者的血浆sdLDL水平(sdLDL占总脂蛋白的百分比)显著高于C等位基因携带者(T/C或C/C)(1.7%比0.8%,P<0.05)㊂表1㊀影响sdLDL水平的基因多态性染色体基因SNP SNPs(个) 11q23.3APOA5/A4/C3/A1rs96418412 19q13.32APOE/C1/C4/C2rs44206386 19q13.32TOMM40rs20756503 1p13.3PSRC1/CELSR2/SORT1rs66024010 11q23.3BUD13rs658956418 11q23.3ZNF259rs20752905 8q24.13TRIB1rs298085338 2p23.3GCKR rs12603263 19q13.32PVRL2rs72548921 2p23-2p24APOB rs56233824 11q23-q24PCSK7rs5084871 19p13.2BCAM rs48037601 7q11.23MLXIPL rs11789773 7q11.23BAZ1B rs69769302㊀㊀注:sdLDL:小而密低密度脂蛋白;SNP:单核苷酸多态性2㊀sdLDL与心血管疾病2.1㊀sdLDL与不同CVD的关系大量研究已证实sdLDL是动脉粥样硬化性CVD的独立危险因素㊂Ikezaki[21]等开展的Framingham Offspring研究显示,sdLDL是最致AS的脂蛋白颗粒㊂此外,对既往无CVD 的受试者进行5年随访后评估颈动脉内中膜厚度(carotid intima media thickness,cIMT)[22],发现sdLDL水平的升高与cIMT进展有更强的相关性(P=0.009)㊂一项分析sdLDL与颈动脉斑块稳定性的研究显示,不稳定斑块组的sdLDL水平高于稳定斑块组(P<0.05)[23]㊂Balling[24]等对38319名普通人群随访3.1年,发现高水平的sdLDL与缺血性卒中(ischemic stroke,IS)的风险增加密切相关,并且调整其他危险因素后,sdLDL高水平组比低水平组发生IS的相对风险升高79%(HR=1.79)㊂Hoogeveen等[19]开展的一项评估社区动脉粥样硬化风险(ARIC)的前瞻性研究中发现,血浆sdLDL 水平与导致AS的脂质谱密切相关,调整已知危险因素后,sdLDL与冠心病(coronary heart disease,CHD)的发生仍具有相关性,其高水平组较低水平组致CHD的相对风险升高51%(HR=1.51)㊂Duran等[8]发现心肌梗死(myocardial infarction,MI)患者的sdLDL水平明显高于对照组,血浆sdLDL水平与MI存在显著相关性(P<0.001),认为sdLDL 水平可能通过增加致死性和非致死性MI的风险影响总体CVD事件的进展㊂国内有关sdLDL与CHD患者冠状动脉病变严重程度㊁经皮冠状动脉介入(percutaneous coronary intervention,PCI)手术预后评估等已有大量报道,通过Gensini评分评估冠状动脉狭窄程度后发现,sdLDL水平与Gensini评分呈正相关,CHD严重程度随着sdLDL水平的升高而显著增加,术前高水平的sdLDL是CHD患者PCI术后发生CVD事件的独立危险因素[25-26]㊂因此,sdLDL是一种与动脉粥样硬化性CVD病情及预后密切相关的新型生物标志物㊂2.2㊀sdLDL致AS机制AS始于胆固醇在内皮下沉积形成脂滴,是CVD事件的主要根源㊂sdLDL被认为是AS中胆固醇沉积的主要来源㊂与LDL相比,sdLDL的体积小,对动脉内膜的穿透力更强㊂sdLDL与LDL受体的亲和力更低㊁血浆半衰期更长㊁对氧化应激更敏感[21]㊂因此,sdLDL比LDL有更强的致AS能力㊂sdLDL致AS的机制包括:sdLDL的颗粒小,易穿透内皮间隙,易与动脉壁中蛋白多糖结合并沉积在动脉壁上,使sdLDL在循环中停留更久,更容易发生致AS性修饰,如氧化㊁脱烷基化和糖基化[27],进一步增强其致AS能力: (1)sdLDL穿透受损的内皮进入内膜时,受损的内皮细胞表达黏附分子,诱导单核细胞向内皮富集,单核细胞进入内膜后释放氧自由基氧化sdLDL㊂经氧化修饰的sdLDL (oxidized,oxsdLDL)在颗粒表面产生氧化特异性表位,吸引更多的单核细胞聚集,单核细胞在内皮下分化为巨噬细胞时,其表面表达清道夫受体(如CD36,SRBI)以识别并吞噬oxsdLDL,转变成泡沫细胞并在内皮下沉积形成脂滴,泡沫细胞释放促炎因子,引起内皮损伤[28]㊂(2)脱烷基化使sdLDL 的唾液酸含量降低,增加sdLDL与动脉壁中蛋白多糖的亲和力,延长sdLDL在动脉壁上的停留时间,促进其在内皮下沉积[22]㊂(3)糖基化使sdLDL颗粒表面的ApoB100发生构象改变,ApoB100在颗粒表面暴露减少导致其与LDL受体亲和力降低,sdLDL不易被LDL受体摄取,在血液中清除缓慢㊁对血管内皮造成持久的损伤[29]㊂此外,sdLDL内抗氧化剂成分如谷胱甘肽㊁过氧化物酶㊁辅酶Q10㊁维生素E及维生素C等含量较少,对氧化应激的抵抗力弱,易被氧化修饰[13]㊂sdLDL内富含脂蛋白磷脂酶A2,其裂解氧化的磷脂时产生促炎因子,加重内皮损伤[30]㊂另外,sdLDL引起的内皮功能障碍进一步激活纤溶系统并产生纤溶酶原激活物抑制剂1和血栓素A2,诱导血栓形成,从而加快AS进展[31](图2)㊂2.3㊀sdLDL与心血管相关代谢性疾病的关系代谢性疾病主要以糖脂代谢异常为特征,是CVD发生及进展的重要危险因素㊂脂质三联征(或致AS脂蛋白表型)是以sdLDL㊁TG升高,高密度脂蛋白(high density lipoprotein,HDL)水平降低为特征,是代谢综合征和2型糖尿病患者血脂异常的表现形式[32]㊂图2㊀sdLDL在循环中的修饰导致动脉粥样硬化㊀㊀sdLDL水平的升高还与代谢性疾病的病理机制有关㊂胰岛素抵抗是肥胖㊁代谢综合征及2型糖尿病患者最常见的代谢紊乱状态㊂生理状态下,胰岛素通过抑制激素敏感性脂肪酶活性,抑制脂肪组织中的脂肪分解,减少游离脂肪酸释放入血㊂此外,胰岛素刺激循环中的LPL及HL活性,加速VLDL等富含TG的脂蛋白水解㊂而在胰岛素抵抗状态下, LPL及HL分解VLDL-TG的作用受抑制,使循环中的VLDL 水平升高㊂另外,HSL抑制脂肪分解的活性受抑制,游离脂肪酸释放入血,使肝脏合成TG增加并分泌更多的VLDL,从而促进sdLDL的生成[7,18]㊂Nakano等[33]发现,糖尿病合并代谢综合征患者的主要表现为高水平sdLDL和低水平HDL,并指出代谢综合征是血浆sdLDL水平升高的重要决定性因素㊂此外,Hoogeveen等[19]研究发现sdLDL水平与体质指数的增加以及糖尿病㊁高血压和代谢综合征等疾病的患病风险性增加有关㊂总之,随着sdLDL水平升高,代谢性疾病患者CVD风险性增加㊂因此,降低sdLDL水平可以降低长期CVD的发生风险㊂3㊀sdLDL与降脂治疗措施血脂异常是引起AS的主要原因之一,因此改善脂质谱已成为抗AS的重要措施㊂临床上常用于治疗血脂异常的药物如他汀类,主要通过抑制羟甲基戊二酰辅酶A还原酶从而减少胆固醇的合成㊂Ishii等[34]将CHD患者随机分为高剂量他汀治疗组和常规剂量他汀治疗组,分别给予高剂量和常规剂量的匹伐他汀,随访1月及6月后评估血浆sdLDL水平,发现两组均能降低sdLDL水平,而高剂量组降低sdLDL 的效果更为明显㊂研究者选择50例已接受他汀类药物治疗的2型糖尿病患者并分为两组,一组应用非诺贝特联合依折麦布治疗,另一组应用他汀类药物治疗,12周后发现与他汀类药物用药组相比,非诺贝特联合依折麦布联合用药组可有效降低sdLDL水平㊂另外,一些新型降脂药物如PCSK9抑制剂也能降低sdLDL浓度CVD高危患者的血浆PCSK9和sdLDL水平呈正相关㊂因此,靶向抑制PCSK9也是降低循环sdLDL水平的一种有前途的策略㊂另一项有关阿利西尤单抗的Ⅱ期临床研究的事后分析结果显示,阿利西尤单抗能显著降低sdLDL水平㊂此外,限制热量摄入㊁体育锻炼㊁减轻体重㊁食用乳制品㊁鳄梨㊁开心果㊁玉米油㊁膳食纤维及ω-3脂肪酸等也是降低sdLDL水平非药物治疗的有效手段[5]㊂因此,应多提倡除药物治疗外通过合理膳食㊁运动及减少体重来降低sdLDL水平,从而降低CVD的风险㊂4㊀小结综上所述,sdLDL因小颗粒㊁低LDL受体亲和力及易被修饰等特征,较其他血脂成份有更强的致AS能力,与CVD 有更强相关性,还在CVD相关代谢性疾病的发生及转归中发挥重要作用㊂因此,sdLDL作为一种强致AS特性的脂蛋白,为基于抗AS靶点的新药研发奠定了基础,开启了降血脂抗AS精准治疗的新思路和新方向,在CVD高危人群的风险评估㊁早期诊断及降低疾病进展具有全新的预测及指导价值㊂鉴于遗传因素影响血浆sdLDL水平,基因检测有望为sdLDL的降脂措施提供新的参考㊂未来,根据影响血浆sdLDL水平的基因多态性的表达与呈现情况,个性化制定治疗方案可能突破新药研发瓶颈,实现CVD的精准治疗㊂利益冲突:无参㊀考㊀文㊀献[1]Li S,Liu Z,Joseph P,et al.Modifiable risk factors associatedwith cardiovascular disease and mortality in China:a PUREsubstudy[J].Eur Heart J,2022,43(30):2852-2863.DOI:10.1093/eurheartj/ehac268.[2]O donoghue ML,Giugliano RP,Wiviott SD,et al.Long-TermEvolocumab in Patients With Established AtheroscleroticCardiovascular Disease[J].Circulation,2022,146(15):1109-1119.DOI:10.1161/CIRCULATIONAHA.122.061620. [3]Fisher WR.Heterogeneity of plasma low density lipoproteinsmanifestations of the physiologic phenomenon in man[J].Metabolism,1983,32(3):283-291.DOI:10.1016/0026-0495(83)90194-4.[4]National Cholesterol Education Program Expert Panel on DetectionE,Treatment of High Blood Cholesterol In A.Third Report of theNational Cholesterol Education Program(NCEP)Expert Panel onDetection,Evaluation,and Treatment of High Blood Cholesterolin Adults(Adult Treatment PanelⅢ)final report[J].Circulation,2002,106(25):3143-3421.DOI[5]Santos HO,Earnest CP,Tinsley GM,et al.Small dense low-density lipoprotein-cholesterol(sdLDL-C):Analysis,effects oncardiovascular endpoints and dietary strategies[J].ProgCardiovasc Dis,2020,63(4):503-509.DOI:10.1016/j.pcad.2020.04.009.[6]Kim HK,Hong J,Ahn S,et al.Association between measured orcalculated small dense low-density lipoprotein cholesterol andoxidized low-density lipoprotein in subjects with or without type2diabetes mellitus[J].J Clin Lab Anal,2023,37(1):e24807.DOI:10.1002/jcla.24807.[7]Vekic J,Zeljkovic A,Stefanovic A,et al.Obesity anddyslipidemia[J].Metabolism,2019,92:71-81.DOI:10.1016/j.metabol.2018.11.005.[8]Duran EK,Aday AW,Cook NR,et al.Triglyceride-RichLipoprotein Cholesterol,Small Dense LDL Cholesterol,andIncident Cardiovascular Disease[J].J Am Coll Cardiol,2020,75(17):2122-2135.DOI:10.1016/j.jacc.2020.02.059. [9]Hwang HW,Yu JH,Jin YJ,et al.Correlation between the smalldense LDL level and nonalcoholic fatty liver disease:Possibility ofa new biomarker[J].Medicine(Baltimore),2020,99(28):e21162.DOI:10.1097/MD.0000000000021162. [10]Krauss RM,Burke DJ.Identification of multiple subclasses ofplasma low density lipoproteins in normal humans[J].J LipidRes,1982,23(1):97-104.[11]Austin MA,Breslow JL,Hennekens CH,et al.Low-densitylipoprotein subclass patterns and risk of myocardial infarction[J].JAMA,1988,260(13):1917-1921.DOI:10.1001/jama.1988.03410130125037.[12]Hoefner DM,Hodel SD,O'brien JF,et al.Development of arapid,quantitative method for LDL subfractionation with use ofthe Quantimetrix Lipoprint LDL System[J].Clin Chem,2001,47(2):266-274.[13]Qiao YN,Zou YL,Guo SD.Low-density lipoprotein particles inatherosclerosis[J].Front Physiol,2022,13:931931.DOI:10.3389/fphys.2022.931931.[14]Hirano T,Ito Y,Saegusa H,et al.A novel and simple methodfor quantification of small,dense LDL[J].J Lipid Res,2003,44(11):2193-2201.DOI:10.1194/jlr.D300007-JLR200. [15]Srisawasdi P,Chaloeysup S,Teerajetgul Y,et al.Estimation ofplasma small dense LDL cholesterol from classic lipid measures[J].Am J Clin Pathol,2011,136(1):20-29.DOI:10.1309/AJCPLHJBGG9L3ILS.[16]Sampson M,Wolska A,Warnick R,et al.A New EquationBased on the Standard Lipid Panel for Calculating Small DenseLow-Density Lipoprotein-Cholesterol and Its Use as a Risk-Enhancer Test[J].Clin Chem,2021,67(7):987-997.DOI:10.1093/clinchem/hvab048.[17]Berneis KK,Krauss RM.Metabolic origins and clinicalsignificance of LDL heterogeneity[J].J Lipid Res,2002,43(9):1363-1379.DOI:10.1194/jlr.r200004-jlr200. [18]Hirano T.Pathophysiology of Diabetic Dyslipidemia[J].JAtheroscler Thromb,2018,25(9):771-782.DOI:10.5551/jat.RV17023.[19]Hoogeveen RC,Gaubatz JW,Sun W,et al.Small dense low-density lipoprotein-cholesterol concentrations predict risk forcoronary heart disease:the Atherosclerosis Risk In Communities(ARIC)study[J].Arterioscler Thromb Vasc Biol,2014,34(5):1069-1077.DOI:10.1161/ATVBAHA.114.303284. [20]Tsuzaki K,Kotani K,Sano Y,et al.The association of the Clock3111T/C SNP with lipids and lipoproteins including small denselow-density lipoprotein:results from the Mima study[J].BMCMed Genet,2010,11:150.DOI:10.1186/1471-2350-11-150.[21]Ikezaki H,Lim E,Cupples LA,et al.Small Dense Low-DensityLipoprotein Cholesterol Is the Most Atherogenic LipoproteinParameter in the Prospective Framingham Offspring Study[J].JAm Heart Assoc,2021,10(5):e019140.DOI:10.1161/JAHA.120.019140.[22]Ikezaki H,Furusyo N,Yokota Y,et al.Small Dense Low-Density Lipoprotein Cholesterol and Carotid Intimal MedialThickness Progression[J].J Atheroscler Thromb,2020,27(10):1108-1122.DOI:10.5551/jat.54130. [23]Pan Z,Guo H,Wang Q,et al.Relationship between subclasseslow-density lipoprotein and carotid plaque[J].Transl Neurosci,2022,13(1):30-37.DOI:10.1515/tnsci-2022-0210. [24]Balling M,Nordestgaard BG,Varbo A,et al.Small dense LDLcholesterol and ischemic stroke[J].Ann Neurol,2023,93(5):952-964.DOI:10.1002/ana.26598.[25]朱竞,王舒,游莉.血清sdLDL-C对冠心病PCI手术患者病情与预后评估的临床价值[J].武汉大学学报(医学版),2021,42(6):1012-1015,1022.DOI:10.14188/j.1671-8852.2019.0476.㊀Zhu J,Wang S,You L.Clinical value of serum sdLDL-C inevaluating the condition and prognosis of patients with coronaryheart disease undergoing PCI[J].Med J Wuhan Univers,2021,42(6):1012-1015,1022.DOI:10.14188/j.1671-8852.2019.0476.[26]孙佳音,闫振娴,郭永和,等.小而密低密度脂蛋白胆固醇与35岁以下青年冠心病患者冠状动脉病变严重程度的关系[J].中国医药,2021,16(8):1135-1139.DOI:10.3760/j.issn.1673-4777.2021.08.004.㊀Sun JY,Yan ZX,Guo YH,et al.Association between smalldense low-density lipoprotein cholesterol and the severity ofcoronary artery lesion among young coronary atherosclerotic heartdisease patients below35years old[J].Chin Med,2021,16(8):1135-1139.DOI:10.3760/j.issn.1673-4777.2021.08.004.[27]Higashioka M,Sakata S,Honda T,et al.Small Dense Low-Density Lipoprotein Cholesterol and the Risk of Coronary HeartDisease in a Japanese Community[J].J Atheroscler Thromb,2020,27(7):669-682.DOI:10.5551/jat.51961. [28]Khatana C,Saini NK,Chakrabarti S,et al.Mechanistic Insightsinto the Oxidized Low-Density Lipoprotein-InducedAtherosclerosis[J].Oxid Med Cell Longev,2020,2020:5245308.DOI:10.1155/2020/5245308.[29]Vekic J,Zeljkovic A,Cicero AFG,et al.AtherosclerosisDevelopment and Progression:The Role of Atherogenic Small,Dense LDL[J].Medicina(Kaunas),2022,58(2):299.DOI:10.3390/medicina58020299.[30]Santoso A,Heriansyah T,Rohman MS.Phospholipase A2is anInflammatory Predictor in Cardiovascular Diseases:Is there anySpacious Room to Prove the Causation?[J].Curr Cardiol Rev,2020,16(1):3-10.DOI:10.2174/1573403x15666190531111932.[31]Jin X,Yang S,Lu J,et al.Small,Dense Low-DensityLipoprotein-Cholesterol and Atherosclerosis:Relationship andTherapeutic Strategies[J].Front Cardiovasc Med,2021,8:804214.DOI:10.3389/fcvm.2021.804214.[32]Vekic J,Zeljkovic A,Stefanovic A,et al.Novel Pharmaceuticaland Nutraceutical-Based Approaches for Cardiovascular DiseasesPrevention Targeting Atherogenic Small Dense LDL[J].Pharmaceutics,2022,14(4):825.DOI:10.3390/pharmaceutics14040825.[33]Nakano S,Kuboki K,Matsumoto T,et al.Small,dense LDLand high-sensitivity C-reactive protein(hs-CRP)in metabolicsyndrome with type2diabetes mellitus[J].J AtherosclerThromb,2010,17(4):410-415.DOI:10.5551/jat.1891.[34]Ishii J,Kashiwabara K,Ozaki Y,et al.Small Dense Low-Density Lipoprotein Cholesterol and Cardiovascular Risk in Statin-Treated Patients with Coronary Artery Disease[J].J AtherosclerThromb,2022,29(10):1458-1474.DOI:10.5551/jat.63229.(收稿日期:2023-03-19)(本文编辑:李鹏)。
Calabi-Yau代数的扩张和形变的开题报告

Calabi-Yau代数的扩张和形变的开题报告开题报告:Calabi-Yau代数的扩张和形变1. 研究背景和意义Calabi-Yau代数是代数学中重要的研究对象。
它被广泛应用于现代理论物理、代数几何和数学物理中,特别是在弦理论、镜像对称和量子场论等领域。
Calabi-Yau 代数的重要性在于它们具有特殊的对称性和拓扑性质,这些性质在以上领域中都有广泛的应用。
在形变理论中,扩张和形变是基本概念。
在 Calabi-Yau 代数的研究中,扩张和形变的研究也具有重要意义。
通过对 Calabi-Yau 代数的扩张和形变的研究,可以深入了解Calabi-Yau 代数的性质,扩展其应用范围,为其他领域提供理论支持。
2. 研究内容本研究将主要包括以下两个方面:(1)Calabi-Yau代数的扩张通过对 Calabi-Yau 代数的扩张研究,可以得到一类新的 Calabi-Yau 代数。
这类新的 Calabi-Yau 代数可能会具有更好的对称性和拓扑性质,然后再应用于其他领域中。
本研究将集中研究Calabi-Yau代数的扩张,主要包括扩张的定义、分类和性质等方面,构建新的扩张模型。
(2)Calabi-Yau代数的形变在 Calabi-Yau 代数的形变研究中,主要关注的是 Calabi-Yau 代数在具有特殊条件下的变化规律。
形变的研究可以扩展 Calabi-Yau 代数的应用范围,并且形变后的 Calabi-Yau 代数也可能具有更好的性质。
本研究将集中研究Calabi-Yau代数的形变,主要包括形变的定义、分类和性质等方面,探讨形变后的新型 Calabi-Yau 代数的特点。
3. 研究方法本研究将采用代数学和拓扑学的基本理论,结合计算机仿真技术,进行Calabi-Yau代数扩张和形变的研究。
主要采用的方法包括扩张论、形变论、同调代数、李代数等基本代数工具及相关算法、数据结构,以及基于MATLAB等计算机仿真软件的数据分析和数值模拟。
古籍参考文献标准格式

古籍参考文献标准格式古籍参考文献标准格式我们在撰写论文的时候,我们都会参考一些文献。
那么你知道古籍参考文献标准格式是什么吗?以下是小编整理的古籍参考文献标准格式,欢迎阅读。
一、参考文献著录格式1 、期刊作者.题名〔J〕.刊名,出版年,卷(期)∶起止页码2、专著作者.书名〔M〕.版本(第一版不著录).出版地∶出版者,出版年∶起止页码3、论文集作者.题名〔C〕.编者.论文集名,出版地∶出版者,出版年∶起止页码4 、学位论文作者.题名〔D〕.保存地点.保存单位.年份5 、专利文献题名〔P〕.国别.专利文献种类.专利号.出版日期6、标准编号.标准名称〔S〕7、报纸作者.题名〔N〕.报纸名.出版日期(版次)8 、报告作者.题名〔R〕.保存地点.年份9 、电子文献作者.题名〔电子文献及载体类型标识〕.文献出处,日期二、参考文献类型1.期刊类【格式】[序号]作者.篇名[J].刊名,出版年份,卷号(期号):起止页码.【举例】[1] 王海粟.浅议会计信息披露模式[J].财政研究,2004,21(1):56-58.[2] 夏鲁惠.高等学校毕业论文教学情况调研报告[J].高等理科教育,2004(1):46-52.[3] Heider, E.R.& D.C.Oliver. The structure of color space innaming and memory of two languages [J]. Foreign Language Teaching and Research, 1999, (3): 62 C 67.2.专著类【格式】[序号]作者.书名[M].出版地:出版社,出版年份:起止页码.【举例】[4] 葛家澍,林志军.现代西方财务会计理论[M].厦门:厦门大学出版社,2001:42.[5] Gill, R. Mastering English Literature [M]. London: Macmillan, 1985: 42-45.3.报纸类【格式】[序号]作者.篇名[N].报纸名,出版日期(版次).【举例】[6] 李大伦.经济全球化的重要性[N]. 光明日报,1998-12-27(3).[7] French, W. Between Silences: A Voice from China[N]. Atlantic Weekly, 1987-8-15(33).4.论文集【格式】[序号]作者.篇名[C].出版地:出版者,出版年份:起始页码.【举例】[8] 伍蠡甫.西方文论选[C]. 上海:上海译文出版社,1979:12-17.[9] Spivak,G. DCan the Subaltern Speak?‖[A]. In C.Nelson & L. Grossberg(eds.). Victory in Limbo: Imigism [C]. Urbana: University of Illinois Press, 1988, pp.271-313.[10] Almarza, G.G. Student foreign language teacher’s knowledge growth [A]. In D.Freeman and J.C.Richards (eds.). Teacher Learning in Language T eaching [C]. New York: Cambridge University Press. 1996. pp.50-78.5.学位论文【格式】[序号]作者.篇名[D].出版地:保存者,出版年份:起始页码.【举例】[11] 张筑生.微分半动力系统的不变集[D].北京:北京大学数学系数学研究所, 1983:1-7.6.研究报告【格式】[序号]作者.篇名[R].出版地:出版者,出版年份:起始页码.【举例】[12] 冯西桥.核反应堆压力管道与压力容器的LBB分析[R].北京:清华大学核能技术设计研究院, 1997:9-10.7.条例【格式】[序号]颁布单位.条例名称.发布日期【举例】[15] 中华人民共和国科学技术委员会.科学技术期刊管理办法[Z].1991―06―058.译著【格式】[序号]原著作者. 书名[M].译者,译.出版地:出版社,出版年份:起止页码.古籍版式介绍凡以前记录古代文献之书籍,通称为古籍。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
with the appropriate projection in the CFTs. This is because D4 , E6 , E8 simple singularities are defined by x3 + xy 2 = 0, x3 + y 4 = 0, x3 + y 5 = 0, up to quadratic terms. We can use Gepner’s 1
hep-th/0010002 UT-908 September 2000
Singular Calabi-Yau Manifolds and ADE Classification of CFTs
arXiv:hep-th/0010002v1 30 Sep 2000
Michihiro Naka
∗
and Masep-th.phys.s.u-tokyo.ac.jp email: nozaki@hep-th.phys.s.u-tokyo.ac.jp
1
Introduction
Currently the study of superstring theory on singular Calabi-Yau manifolds is active. The
for the singular Calabi-Yau compactification in terms of the spectral flow invariant orbits of the tensor product theories with the theta function which encodes the momentum mode of the Liouville theory. Furthermore we find the interesting identity among characters in minimal models at different levels. We give the complete proof for the identity.
important feature of superstrings propagating near singularities is the appearance of light solitons coming from the D-branes wrapped around the vanishing cycles. This is the nonperturbative quantum effect in string theory in the sense that even after taking gs → 0, the VEV of dilaton will blow up at the singular point. In order to appear such an effect, the vanishing worldsheet theta angle is necessary [1], which seems to make worldsheet CFTs singular [2]. In contrast to the well-established perturbative description of smooth Calabi-Yau manifolds like Gepner models [3], the worldsheet description of singular Calabi-Yau manifolds remains to be investigated. This situation is expected to bring us the new source of insights of stringy dynamics related to the space-time singularity. Moreover, this set up only depends on the type of the singularity, and has an interesting physical application that the decoupled theory in this background corresponds to, for example, four-dimensional CFTs classified by ADE [4]. Such a CFT can be described by the N = 2 Landau-Ginzburg model with a superpotential including a negative power of some chiral superfield in order to push up the central charge to the right value for Calabi-Yau manifolds, and this peculiar term was handled by Kazama-Suzuki model [5] for the non-compact coset SL(2, R)/U (1) [6, 7]. Recently, after the renowned AdS/CFT correspondence [8], the approach based on a holographic point of view was proposed [9]. In this approach, the sector of Landau-Ginzburg theory with a negative power superpotential is replaced by Liouville theory [10]. This Liouville field corresponds to an extra non-compact direction, which indicates holography. For this description, the first consistency check is to make the modular invariant partition function on a torus, and to see that the partition function vanishes. This issue was systematically pursued in [7, 11] for the singular Calabi-Yau manifold with an isolated ADE singularity (or conifold [12]). Furthermore, the extension to more complicated singularities was made [13]. In all the case, the string theory on the Calabi-Yau manifold with the ADE singularity is treated as N = 2 minimal model classified by ADE and Liouville theory. Now, we wish to pose the point of view taken throughout this paper. In the LandauGinzburg description of N = 2 minimal models, the following relation should hold [14]: D4 = A2 ⊗ A2 , E6 = A2 ⊗ A3 , E8 = A2 ⊗ A4 , (1)
construction [3] for the tensor product theory in order to reproduce the feature of block diagonal D4 , E6 , E8 modular invariants. At first sight, one may think that D4 invariant is not written by the tensor product theory due to the term xy 2 in the above polynomial. However we can rewrite the polynomial into the form x3 + y 3 by the suitable linear transformation of variables. Above relation (1) can be reduced to the identity among characters in minimal models at different levels. In principle, this can be predicted by the comparison of weight and U (1) charge of the irreducible representations in minimal models. But the physical meaning has been lacking. The partition function for singular Calabi-Yau manifolds is the slight generalization of well-known ADE classification of modular invariant (for the recent discussion, see [15]). If this partition function reflects the singularity of spacetime, there shold exist the reformulation of D4 , E6 , E8 invariants by the tensor product theory as in (1) and the identity among characters in minimal models. This is our interpretation of the conventional simple singularity. However, we cannot proceed the other non-diagonal modular invariants Dn>4 , E7 . In order to make modular invariant of the tensor product theory, we use the so-called spectral flow method [16] which makes the block diagonalization and the spacetime supersymmetry manifest. One reason for using the spectral flow method is that for singular K3 surface, we can reproduce the block diagonal elements of D4 , E6 , E8 modular invariants as the spectral flow invariant orbits by the tensor product theory of suitable minimal models. This cannot be seen only with the minimal models. Due to the identity among the characters of minimal models, the extension to the singular Calabi-Yau 3 or 4-folds is straightforward. Finally we give the proof of the proposed identity. The paper is organized as follows. In section 2, we briefly review the construction of the modular invariant partition function in the system of superstrings on Calabi-Yau manifolds with the isolated ADE singularity, based on the Liouville system and minimal model classified by ADE. Section 3 is devoted to introduce the necessary background and notation about the spectral flow method used in the remainder of the paper. In section 4, we propose our main results about the D4 , E6 , E8 modular invariants. We explicitly identify the block diagonal elements of D4 , E6 , E8 invariants by the spectral flow invariant orbits of Gepner models of different levels. Section 5 includes the conclusion and discussion. In the Appendix A, we A2 ⊗ A2 , A2 ⊗ A3 , A2 ⊗ A4 and derive the identity among characters in minimal models at
