第四章、应变疲劳
疲劳计算影响因素

影响疲劳寿命分析的因素
材料(material)
载荷(loading) 定义(definition) 分析选项(analytical options) 寿命单位(life unit)
材料(material)
疲劳强度因子(fatigue strength factor)
疲劳分析计算完毕后,根据疲劳强度因子Kf修正 应力或者应变疲 劳曲线。 This setting is used to account for a "real world" environment that may be harsher than a rigidly-controlled laboratory environment in which the data was collected。Common fatigue strength reduction factors to account for such things as surface finish can be found in design handbooks。
疲劳寿命分析方法——名义应力有限寿命设计法
有限寿命设计法常常成为安全寿命设计法。它是无限寿命设计法 的直接发展。两者的基本参数都是名义应力,其设计思想也大体 相似,都是从材料的S-N曲线出发,考虑各种因素影响,得出零件 的S-N曲线,并根据零件的S-N曲线进行疲劳设计。
所不同的是,有限寿命设计法使用的是S-N曲线的左支——斜线部 分,亦即有限寿命部分。另外,由于斜线部分的疲劳寿命各不相 同,因此在对材料的S-N曲线进行修正时,要考虑循环数对各影响 系数的影响。
另外,无限寿命的设计应力都低于疲劳极限,因此比设计力低 的低应力对零件的疲劳强度没有影响,设计计算时不管实际的工 作应力如何变化,只需要按照最高应力进行强度校核即可。
应力疲劳法,应变疲劳法,断裂疲劳法

应力疲劳法,应变疲劳法,断裂疲劳法应力疲劳法、应变疲劳法和断裂疲劳法是材料科学和工程领域中常用的疲劳试验方法。
这些方法可用于评估材料在长期重复加载下的疲劳性能,以及预测材料的寿命。
下面将分别介绍这三种疲劳试验方法及其应用。
一、应力疲劳法应力疲劳法是通过施加周期性的应力加载来评估材料的疲劳性能。
在应力疲劳试验中,材料会在一定的应力水平下进行重复加载,加载过程中记录应力和应变数据。
通过分析应力-应变曲线,可以得到材料的疲劳寿命和疲劳强度。
应力疲劳法可以用于评估金属材料、复合材料和橡胶等各种材料的疲劳性能。
二、应变疲劳法应变疲劳法是通过施加周期性的应变加载来评估材料的疲劳性能。
在应变疲劳试验中,材料会在一定的应变幅值下进行重复加载,加载过程中记录应力和应变数据。
通过分析应力-应变曲线,可以得到材料的疲劳寿命和疲劳强度。
应变疲劳法在评估纤维增强复合材料等材料的疲劳性能时,具有一定的优势。
三、断裂疲劳法断裂疲劳法是通过施加循环加载并观察材料破裂的方式来评估材料的疲劳性能。
在断裂疲劳试验中,材料会在一定的加载循环数下进行重复加载,加载过程中记录应力和位移等数据。
通过分析应力-位移曲线,可以得到材料的疲劳寿命和疲劳强度。
断裂疲劳法适用于评估金属材料、混凝土和岩石等材料的疲劳性能。
这三种疲劳试验方法在实际工程中有着广泛的应用。
例如,在航空航天领域,疲劳性能是评估飞机部件和发动机部件可靠性的重要指标之一。
通过应力疲劳法、应变疲劳法和断裂疲劳法,可以对材料在复杂载荷下的疲劳行为进行研究,提高航空器的安全性和可靠性。
疲劳试验方法还可以应用于材料的研发和设计过程中。
通过对不同材料的疲劳性能进行评估,可以选择合适的材料用于特定的工程应用,提高产品的寿命和可靠性。
同时,疲劳试验方法也可以用于研究材料的疲劳机制和损伤演化规律,为材料的改进和优化提供科学依据。
应力疲劳法、应变疲劳法和断裂疲劳法是评估材料疲劳性能的重要方法。
这些方法可以通过施加不同的加载方式,对材料的疲劳寿命和疲劳强度进行评估,为工程应用和材料设计提供依据。
疲劳与断裂

变幅载荷
随机载荷
24
Three primary fatigue analysis methods which are the stress-life approach, strainlife approach, and the fracture mechanics approach, will be discussed. These methods have their own region of application with some degree of overlap between them.
二、疲劳破坏机理及断口微观特征
疲劳裂纹萌生机理:
疲劳裂纹的起始或萌生,称为疲劳裂纹成核。 疲劳裂 纹成核 扩展至临 界尺寸 断裂 发生
裂纹起源(裂纹源)在何处? 高应力处: 1)应力集中处;缺陷、夹杂,或孔、切口、台阶等 2)构件表面; 应力较高,有加工痕迹, 平面应力状态,易于滑移发生。
16
延性金属中的滑移
19
疲劳条纹(striation) 不同于海滩条带(beach mark) Cr12Ni2WMoV钢疲劳条纹:(金属学报,85)
透射电镜:1-3万倍
S
谱块
t
循环
条纹
20
条带
疲劳裂纹扩展的微观机理 1976 Crooker
Cr12Ni2WMoV钢疲劳断口微观照片:(金属学报,85)
三种破坏形式:
微解理型 microcleavage
23
1.5 疲劳问题研究方法
裂纹扩展规律 断裂力学规律
缺口影响 尺寸、光洁度 等影响 平均应力的影响 Goodman直线 Miner 累积损伤理论 雨流计数法
损伤容限设计 构件S-N曲线 (各种修正) 无限寿 命设计 安全寿 命设计
疲劳试验

第一步 采用升降法测定条件疲劳极限, 第二步 用成组法测定σ一N曲线有限寿命段上各 点的数据, 第三步 绘制σ一N曲线。 二、不同应力状态下的疲劳极限 根据大量的实验结果,弯曲与拉压、扭转疲劳 极限之间的关系: 钢:σ-1p=0.85σ-1,铸铁σ-1p=0.65σ-1 铜及轻合金:τ-1=0.55σ-1,铸铁τ-1=0.8σ-1 σ-1>σ-1p>τ-1
2、特点
(1)断裂应力<σb,甚至<σs;
(2)出现脆性断裂;
(3)对材料的缺陷十分敏感;
(4)疲劳破坏能清楚显示裂纹的萌生和扩展, 断裂。
三、疲劳曲线和疲劳极限
(一)疲劳曲线
1、对称循环疲劳曲线 (σ~N曲线)
P96-图5-3
(1)有水平段的疲劳 曲线(钢) (2)无水平段的疲劳 曲线(有色金属,不锈钢等)
若为韧性材料max023公式法上两图中的曲线可用数学公式表示可以很方便利用02和r求得第二节疲劳抗力指标及其测定二不同应力状态下的疲劳极限根据大量的实验结果弯曲与拉压扭转疲劳极限之间的关系
第四章 疲劳试验
引言
材料构件在变动应力和应变的长期作用下, 由于累积损伤而引起的断裂的现象——疲劳。 疲劳属低应力循环延时断裂。 不产生明显的塑性变形,呈现突然的脆断。 ∴疲劳断裂是一种非常危险的断裂。 ∴工程中研究疲劳的规律、机理、力学性能指 标、影响因素等,就具有重要的意义。
(2)σmax~σm 图 y轴上的边界点为σ-1和 -σ-1,x轴则同前图。 σmax=σb ,利用不同的 应力比r来作图。若为韧性 材料σmax=σ0.2 (3)公式法 上两图中的曲线可用数学 公式表示 可以很方便利用 σb ,σ-1, σ0.2和r,求得σr。
第二节 疲劳抗力指标及其测定 一、疲劳极限的测定
第四章应变疲劳
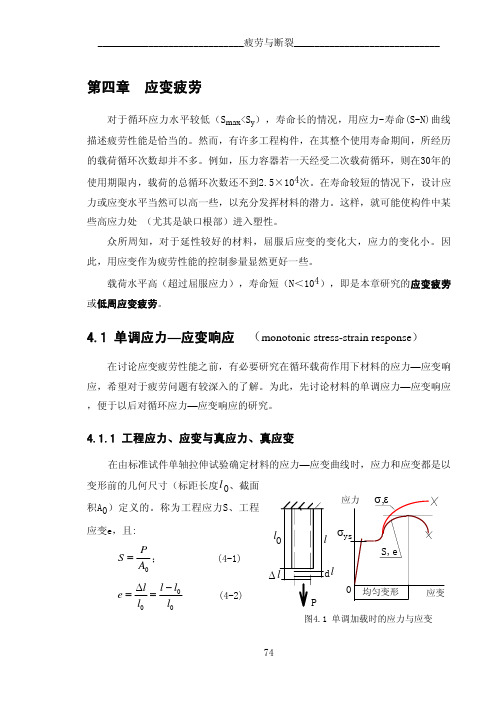
大多数金属材料,在循环数增加到一定次数后,σ-ε响应逐渐趋于稳定,形成 稳态滞后环。图4.3中低碳钢,在N约100次左右,即可形成稳态滞后环。当然,也 有些材料需要较长的循环次数才能形成稳态环;甚至还有些材料的滞后环一直都不 会稳定。对于这些材料,往往用在该应变幅下,一半寿命处的滞后环,作为名义稳 态滞后环。
在讨论应变疲劳性能之前,有必要研究在循环载荷作用下材料的应力—应变响 应,希望对于疲劳问题有较深入的了解。为此,先讨论材料的单调应力—应变响应 ,便于以后对循环应力—应变响应的研究。
4.1.1 工程应力、应变与真应力、真应变
在由标准试件单轴拉伸试验确定材料的应力—应变曲线时,应力和应变都是以
变形前的几何尺寸(标距长度l 0、截面
则由前述各式即有:
σ=P/A=Pl /A0l 0=(P/A0)[(l 0+Δl )/l 0]=S(1+e)
(4-5)
ε=ln(1+e)=ln(l /l 0)=ln(A0/A)=ln[100/(100-RA)]
(4-6)
式中,RA=(A0-A)×100/A0, 是截面收缩率。
上述二式,给出了均匀变形阶段工程应力、应变与真应力、真应变间的关系。
ln( 1 + e )
l 为加载到P时,变形后的长度。
(4-4)
随着载荷增加,材料进入屈服,经过强化、颈缩直至最后断裂。在颈缩之前,
试件发生伸长的同时,其横截面均匀缩小,称为均匀变形阶段,如图4.1所示。
忽略弹性体积变化,假定发生变形后体积不变,则在颈缩之前的均匀变形阶
段,可以假设有:
A0l 0=Al
4.3.1 材料的记忆特性 σ
图4.6是典型的加载—卸载—加载曲线图。如
应变疲劳
σ ε = εe + ε p = + E K
σ
1n
滞后环
• • •
应力应变响应随循环次数而改变 一定周次后有稳定滞后环 有循环硬化和软化现象
循环应力-应变曲线
• 由不同应变恒幅对称循环控制下的疲劳实验,得到一族 由不同应变恒幅对称循环控制下的疲劳实验,
l
• 真实应变 ε =
应力-应变曲线
塑性材料模型
弹性应力-应变曲线
• 应力与弹性应变关系,Hooke定理 应力与弹性应变关系, • 应力与塑性应变关系,Holomon定理 应力与塑性应变关系, • K为强度系数,n为应变硬化指数 为强度系数,
σ = Eε
σ = K (ε p )
n
应力-应变曲线
作业
• 弹性模量E=2.1e5MPa,K’=1220MPa, n’=0.2,求循环
响应; 响应;
σ 稳态滞后环,即循环 a − ε a 曲线; 稳态滞后环, 曲线; • 与单调 σ − ε不同,选好载荷作用下的循环 σ a − ε a 曲线; 不同, 曲线;
材料记忆特性
• 应变第二次到达某处,该处曾发生过应变反向,形成封 应变第二次到达某处,该处曾发生过应变反向,
闭环; 闭环; • 过封闭环顶点,路径不受封闭环的影响,仍记得原来的 过封闭环顶点,路径不受封闭环的影响, 路径; 路径;
应变疲劳简介
华北电力大学可再生能源ቤተ መጻሕፍቲ ባይዱ院-龙凯 华北电力大学可再生能源学院 龙凯 longkai1978@
工程应力、应变
• 工程应力S=P/A0 • 工程应变e=(l-l0)/l0
真实应力、应变
疲劳与断裂课程,,学习指南

疲劳与断裂课程,,学习指南疲劳与断裂课程学习指南一、教材教育部面向21 世纪课程教材:陈传尧编著,疲劳与断裂,华中科技大学出版社,2002 年。
二、辅助教材王忠光译,S. Suresh(美)著,材料的疲劳,北京:国防工业出版社,1999年第二版郑朝云、张式程译,D. 拉达伊(德)著,焊接结构疲劳强度,北京:机械工业出版社,1994 年第一版熊俊江著,疲劳断裂可靠性工程学,北京:国防工业出版社,2008 年第一版三、教学内容疲劳与断裂课程共分10 章。
第一章绪论;第二、三和四章介绍疲劳裂纹萌生及其研究方法,包括高周应力疲劳和低周应变疲劳,以及疲劳问题研究的统计学基础;第五、六和七章介绍弹塑性断裂力学基础,包括断裂扩展判据、断裂控制设计方法,以及工程常见的表面裂纹的应力强度因子;第八、九和十章介绍疲劳裂纹扩展的研究和预测方法。
各章主要内容如下。
第一章绪论,介绍疲劳的基本概念,疲劳断裂破坏事故的严重性,疲劳设计的主要方法和发展历史,疲劳破坏的特征和机理,疲劳断裂问题研究的一般方法。
第二章应力疲劳,介绍应力疲劳的基本概念,S-N 曲线及其近似估计,平均应力、载荷形式、尺寸效应、结构件表面光洁度、表面处理,以及温度与环境等对材料疲劳性能的影响,在给定寿命下循环应力幅与平均应力之间的关系,等疲劳寿命图,考虑缺口的疲劳问题,Miner 线性累积损伤理论,变幅载荷谱下的疲劳问题,简化雨流循环计数法,随机载荷谱下的疲劳问题。
第三章疲劳应用统计学基础,介绍疲劳数据的分散性,描述疲劳寿命分布的两种主要分布函数:正态分布和威布尔分布,二元线性回归方法,S-N 曲线和p-S-N 曲线的拟合,以及利用回归方程进行寿命问题的统计推断。
第四章应变疲劳,介绍应变疲劳的基本概念,单调的应力应变响应及其描述,滞后环,循环应力应变响应及其描述,材料的记忆特性,变幅循环应力应变响应计算,应变寿命曲线与平均应力影响,考虑缺口的应变寿命分析。
疲劳与断裂应变疲劳1

3) 材料的循环性能:
循环应力应变曲线
ea=
eea
epa=
a E
(
a K
)1
n
滞后环曲线
De =
Dee
Dep =
D E
2
(
D 2 K
1
)
n
4) 变幅循环下的应力-应变计算方法:
第一次加载,由a-ea曲线描述,已知ea算a。 后续反向,由De-D曲线描述;
由谱中已知的De算相应的D,且有: ei+1 =ei Dei-i+1 ; i+1=i Di-i+1
3-4 卸载。经过2’处时,应变曾在该处 (2处)发生 过反向,由记忆特性知2-3-2’形成封闭环, 且不影响其后的-e响应。
按路径 1-2-4计算-e响应,有:
D
D
De =
1-4 2 (
1-4 ) 1 n
得到: e41=-e41-De1-4;E4=1-D1-4。2 K
e
1 3
1' 5 5'
2 2’ 7
4) 依据计算数据(eI ,i ), 画出-e响应曲线。
例4.1 变幅应变谱如图。已知 E=2.1×105MPa, K'=1220MPa, n'=0.2, 试计算其循环响应。
解:0-1 e1=1/E+(1/K')1/n'
e1=0.01 \1=462MPa
1-2 卸载。 De1-2=D1-2/E+2(D1-2/2K')1/n' De1-2=0.012 \D1-2=812MPa 故:e2=e1-De1-2=-0.02;
Masing效应
在不同应力水平得到的滞回环通过坐标平移,使其最低点与原点 重合,如果滞回环最高点的连线与其上行线重合,则该材料具有 Masing效应。
疲劳与断裂4ppt课件第四章节应变疲劳

多轴应变疲劳研究
总结词
多轴应变疲劳是工程结构中常见的应力 状态,研究多轴应变疲劳对于提高结构 的安全性和可靠性具有重要意义。
VS
详细描述
多轴应变疲劳涉及到多个方向的应力分量 ,其断裂行为和寿命预测比单轴应变疲劳 更为复杂。因此,需要深入研究多轴应变 疲劳的机制和规律,包括多轴应变疲劳的 损伤演化、寿命预测和实验技术等。
THANKS
感谢观看
02
应变疲劳的实验研究
应变疲劳实验方法
实验对象
选择一定数量的受试者,可以是 不同年龄、性别和身体状况的人 群,以探究应变疲劳在不同个体 之间的表现。
实验设计
设计合理的实验方案,包括应变 方式、应变时间、恢复时间等, 确保实验过程科学、严谨。
实验操作
在实验过程中,确保受试者按照 规定的应变方式进行操作,并记 录相关数据。
影响应变疲劳的因素包括材料特性、应力水平、温度、环 境条件等。
要点二
详细描述
材料的特性,如硬度、韧性、强度等,对应变疲劳有显著 影响。高硬度和脆性的材料更容易发生应变疲劳。应力水 平,特别是低应力幅值和高循环应变,也是影响应变疲劳 的重要因素。温度和环境条件,如湿度、腐蚀介质等,也 会对应变疲劳产生影响。在高温和腐蚀环境中,材料的抗 应变疲劳性能通常会降低。
01
根据实验结果分析,总结应变疲劳在不同个体之间的表现和规
律。
提出研究建议
02
根据实验结论,提出对应变疲劳进一步研究的建议和方法,为
相关领域的研究提供参考。
应用前景展望
03
探讨应变疲劳在生产、生活等方面的应用前景,为实际应用提
供指导。
03
应变疲劳的数值模拟
数值模拟方法
应变疲劳实例

应变疲劳实例
应变疲劳是当一个人因受到应力而变得疲惫不堪的一种状态。
它可能会让一个人的身体和心理变得疲惫不堪,最终影响到他们的日常生活以及工作表现。
下面将用一个实例来描述这种应变疲劳状态:杰克(Jack)是一名30岁的职场新人,正在努力地从事他的工作。
由于过于勤奋而疲惫,他经常工作到深夜,睡眠不足,以至于身体和精神都不好,这种情况一直持续了几个月,情况最终恶化到某一天他只能睡到中午才能起床并继续工作。
虽然最终杰克仍然保持良好的工作表现,但是由于他的身体和精神处于应变疲劳的状态,他的工作效率也相应地降低了。
他的记忆力也减弱了,而且他的情绪不稳定,他常常沮丧、抑郁,甚至经常表现出焦虑、恐惧、躁狂以及紧张等不良情绪。
他最终发现,最好的解决办法是开始一些身体和心理的休息,保持睡眠正常,并在工作之外抽出一些时间来进行身体和心理健康的活动。
- 1 -。
第四讲 损伤理论,荷载谱处理,应变疲劳

第四讲损伤理论,荷载谱处理,应变疲劳上节概述p-S-N曲线,失效率,存活率疲劳数据处理,正态分布,标准正态分布检验数据是否服从正态分布,正态分布坐标纸正态分布存在的问题:不能反映构件疲劳寿命有一个大于等于零的下限。
威布尔分布,与正态分布的比较威布尔分布坐标纸回归方程,最小二乘法相关系数,起码值回归分析的基本方法损伤理论疲劳积累损伤理论是构件在变幅疲劳荷载作用下疲劳损伤的积累规则和疲劳破坏的准则。
疲劳积累损伤理论回答下述三个问题1.一个荷载循环对材料或结构造成的损伤是多少?2.多个荷载循环时,损伤是如何积累的?3.失效时的临界损伤是多少?1)线性疲劳积累损伤理论线性疲劳积累损伤理论假设在循环荷载作用下,疲劳损伤是可以线性累加,各应力之间相互独立,当累加损伤到某一数值构件发生疲劳破坏。
Palmgren -Miner (P -M )线性疲劳积累损伤准则若构件在某恒幅应力水平S 作用下的疲劳寿命为N ,则经受n 次循环时的损伤为: Nn D =构件在应力水平S i 作 用下经受n i 次循环的损伤 为D i = n i /N i ,在k 个应力 水平作用下的总损伤为: ∑∑====ki iik i i N n D D 11破坏准则:11==∑=ki iiCR N n DMiner 疲劳积累损伤理论没有考虑荷载作用的先后次序。
材料“锻练效应”(training effect )一般,高-低加载顺序临界积累损伤值D CR 小于1,低-高加载顺序临界积累损伤值D CR 大于1。
N 1 N 2 S 1S 3S maxNS 2N 3变幅拉-压疲劳寿命对于随机荷载,按Miner 理论计算破坏时的临界损伤值D CR 接近于1。
Manson 双线性模型Manson 在修正Miner 准则时提出的两级疲劳加载时的疲劳寿命预测公式η⎪⎪⎭⎫ ⎝⎛-=11221N n N nη:与疲劳加载顺序相关的参数当疲劳加载从高到低时0 < η < 1,反之η > 1。
第四章 材料的疲劳ppt课件

对青铜:
σ-1 =0.21 σb
疲劳极限与材料强度近似成正比,所以合金化、
细化晶粒和组织等强化方法可以提高材料的疲劳
极限。
.
(2)非对称应力循环下的疲劳极限
大多数机械零件所承受载荷属于非对称循 环应力。 ——考虑平均应力、应力幅、应力比
应力比提高, 疲劳极限和 疲劳寿命增 长!
.
平均应力提高,疲劳极限和疲劳寿命减小!
不适用于循环频率较高的试验,故也称低频疲劳或应变疲劳。
.
观察试件在这一阶段的破坏断口,可见到材料已
发生塑性变形的特征。所以低周疲劳性能常用应 变-寿命曲线表征。一般的疲劳曲线特指N>104范
围内的应力-寿命曲线。
有些机械零件,例如一次性使用的火箭发动机的某些零件、 导弹壳体等,在整个使用寿命期间应力变化次数只有几百到 几千次,故其疲劳属于低周疲劳。但对绝大多数通用零件来 说,当其承受变应力作用时,其应力循环次数总是大于 10000的。所以大部分是高周疲劳。
.
例题
疲劳试验的平均应力是50MPa,应力变化 幅度是30MPa。试计算:1、最大应力;2、 最小应力;3、应力比。
解:平均应力σm= (σmax+σmin)/2=50 应力变化Δσ=2σa= (σmax-σmin)=30 σmax=65MPa;σmin=35MPa;r=0.54
.
1.2 疲劳破坏
德国人Wohler针对火车车轴疲劳进行研究, 得到了循环应力(S)与疲劳循环寿命(N) 之间的关系。——疲劳曲线(S-N曲线)
.
旋转弯曲疲劳试验
试样旋转并承受一弯矩。 产生弯矩的力恒定不变且 不转动。试样可装成悬臂, 在一点或两点加力;或装 成横梁,在四点加力。试 验一直进行到试样失效或 超过预定应力循环次数。
Ch04应变疲劳

应变疲劳或低周应变疲劳:
载荷水平高 (>ys),寿命短 (N<104)。
2
4.1 单调应力-应变响应
1. 变量定义
工程应力S: Engineering stress
工程应变e: Engineering strain
15
8-1’ 加载。注意有封闭环7-8-7’, 5-6-5‘, 1-4-1’,故有: 1'=1; 1'=1。
依据计算数据(i ,i ), 在 坐标中描点,顺序连 接,即可得到 响应曲 线。
1 3
1' 5 5'
2 2’ 0
7
7' t
68
4
5 5'
1 1'
7 7'
3
0
采用雨流计数法,可获得与
8
以上分析一致的结果。
S P A0
e l l l0
l0
l0
P
A A0
d0 l0
dl
P
original deformed
材料纵向伸长,横向缩小。真应力、真应变?
3
P
真应力:
P
A
True stress
真应变 true strain
A
l dl
l l0
dl
l0
l
l
dl
且有:
P
deformed
P
l dl ln l l ln l0 l ln(1 e)
0.6 f
(
N
)0.6
Su为极限强度,f是断裂真应 变。仅适用于恒幅对称应变
workbench疲劳分析

第一章简介1.1 疲劳概述构造失效的一个常见原因是疲劳,其造成破坏与重复加载有关。
疲劳通常分为两类:高周疲劳是当载荷的循环〔重复〕次数高(如1e4 -1e9)的情况下产生的。
因此,应力通常比材料的极限强度低,应力疲劳〔Stress-based〕用于高周疲劳;低周疲劳是在循环次数相对较低时发生的。
塑性变形常常伴随低周疲劳,其说明了短疲劳寿命。
一般认为应变疲劳〔strain-based〕应该用于低周疲劳计算。
在设计仿真中,疲劳模块拓展程序〔Fatigue Module add-on〕采用的是基于应力疲劳〔stress-based〕理论,它适用于高周疲劳。
接下来,我们将对基于应力疲劳理论的处理方法进展讨论。
1.2 恒定振幅载荷在前面曾提到,疲劳是由于重复加载引起:当最大和最小的应力水平恒定时,称为恒定振幅载荷,我们将针对这种最简单的形式,首先进展讨论。
否那么,那么称为变化振幅或非恒定振幅载荷。
1.3 成比例载荷载荷可以是比例载荷,也可以非比例载荷:比例载荷,是指主应力的比例是恒定的,并且主应力的削减不随时间变化,这实质意味着由于载荷的增加或反作用的造成的响应很容易得到计算。
相反,非比例载荷没有隐含各应力之间相互的关系,典型情况包括:σ1/σ2=constant在两个不同载荷工况间的交替变化;交变载荷叠加在静载荷上;非线性边界条件。
1.4 应力定义考虑在最大最小应力值σmin 和σmax作用下的比例载荷、恒定振幅的情况:应力X围Δσ定义为(σmax -σmin)平均应力σm 定义为(σmax+σmin)/2应力幅或交变应力σa是Δσ/2应力比R是σmin /σmax当施加的是大小相等且方向相反的载荷时,发生的是对称循环载荷。
这就是σm=0,R=-1的情况。
当施加载荷后又撤除该载荷,将发生脉动循环载荷。
这就是σm =σmax/2,R=0的情况。
1.5 应力-寿命曲线载荷与疲劳失效的关系,采用的是应力-寿命曲线或S-N曲线来表示:〔1〕假设某一部件在承受循环载荷, 经过一定的循环次数后,该部件裂纹或破坏将会开展,而且有可能导致失效;〔2〕如果同个部件作用在更高的载荷下,导致失效的载荷循环次数将减少;〔3〕应力-寿命曲线或S-N曲线,展示出应力幅与失效循环次数的关系。
应变疲劳实例

应变疲劳实例
应变疲劳是指材料在反复受到应力或应变作用后发生的疲劳破
坏现象。
这种现象在我们身边随处可见,比如汽车车轮、飞机发动机叶片、建筑结构等等。
下面将介绍一些应变疲劳的实例。
1. 汽车车轮应变疲劳
汽车车轮是一个常见的应变疲劳实例。
当汽车行驶时,轮胎受到路面的冲击力,车轮也会随之受到应力的作用。
随着行驶里程的增加,车轮会发生微小的变形,这些变形会在不断的叠加下导致车轮的应变疲劳破坏。
如果车轮的应变疲劳程度过高,就会导致车轮的断裂,使得车辆无法正常行驶,甚至发生严重的交通事故。
2. 飞机发动机叶片应变疲劳
飞机发动机叶片也是一个常见的应变疲劳实例。
当飞机在高空飞行时,飞机发动机叶片会受到高速旋转产生的巨大离心力和温度的影响。
这些影响会导致叶片表面产生微小的应变,并在不断的叠加下导致叶片的应变疲劳破坏。
如果飞机发动机叶片的应变疲劳程度过高,就会导致叶片的断裂,使得飞机发动机失灵,从而威胁到乘客的生命安全。
3. 建筑结构应变疲劳
建筑结构也是一个常见的应变疲劳实例。
在风、雨、地震等自然灾害的影响下,建筑结构会受到巨大的应力和应变作用。
这些应力和应变会在结构内部产生微小的变形,并在不断的叠加下导致结构的应变疲劳破坏。
如果建筑结构的应变疲劳程度过高,就会导致结构的倒
塌,使得建筑物内部人员遭受伤害。
综上所述,应变疲劳是一种非常常见的疲劳破坏现象。
了解应变疲劳的实例可以帮助我们更好地认识应变疲劳的危害性,加强材料的疲劳强度设计,从而保障我们的生命安全。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
B B' C D' D
加载ABD, 卸、加载曲线ABCB‟D。
材料记得曾为反向加载所中断 的应力-应变路径。
A
e
材料的记忆规则为: 1) 应变第二次到达某处,该处曾发生过应变反向, 则形成封闭环。 (封闭环B-C-B‟)
2) 过封闭环顶点后,-e路径不受封闭环的影响, 记得原来的路径。原路径A-B-D.
17
反映加载路径的是D-De曲线, D 1- 2 D 1- 2 1 n + 2( ) 即:D e 1- 2 = E 2K
已知De1-2= e1-e2 。可求D1-2; 从 1到 2是卸载,则2处有: e2=e1-De1-2 2=2-D1-2
e
1 3
2 5 2’ 7 7' 6 8 5'
e = ee + e p =
+( ) E K
1n
K为强度系数,应力量纲(MPa);
n为应变硬化指数,无量纲。 n=0,理想塑性材料。
10
4.2 滞后环和循环应力-应变响应
Monotonic stress-strain curves have long been used to obtain design parameters for limiting stress on engineering structures and components subjected to static loading. Similarly, cyclic stress-strain curves are useful for assessing the durability of structures and components subjected to repeated loading. 单调应力--应变曲线长期用于承受静载作用的工程 结构和构件,以获得极限应力设计参数。类似地, 循环应力--应变曲线用于评价承受重复载荷作用的 结构和构件的耐久性。
可见,=S(1+e)>S,相对误差为: (-S)/S=e, 故e越大,(-S)越大。e=0.2%时,比S大0.2%。
e是小量,展开得:e=ln(1+e)=e-e2/2+e3/3-…<e, e比e小,相对误差为: (e-e)/e=e/2。
e<0.01时,与S,e与e相差小于1%,可不加区别。
deformed
S-e
0 均匀变形
应变
8
工程应力、应变与真应力、真应变间关系
在均匀变形阶段,忽略弹性体积变化,假定变 形后体积不变,A0l0=Al,则有关系:
=P/A=Pl/A0l0=(P/A0)[(l0+Dl)/l0]=S(1+e) e=ln(1+e)=ln(l /l0)=ln(A0/A)=ln[100/(100-RA)]
许多构件中关键部位(缺口)的材料响应与应变 或变形相关,应变-寿命方法正是以此为基础的。
2
When load levels are low, stress and strain are 高载荷水平: related. Consequently, in this range, loadlinearly high load level 应力变化小,难于控制; controlled and strain-controlled tested results are 应变变化大,利于控制。 equivalent. At high load levels, in the low cycle fatigue region, thelevel 低载荷水平: response and low load cyclic stress-strain 应力控制和应变控等效。 the material behavior are best modeled under 0 e strain-controlled conditions.
11
4.2 滞后环和循环应力-应变响应
1. 滞后环 hysteresis loops 在ea=const的对称循环下, 应力、应变的连续变化。
a
可知: 0 ea 1)-e响应随循环次数改变。 N=2 稳态环 100 2)一定周次后有稳态滞后环。 低碳钢的循环应力应变响应 3)有循环硬化和软化现象。
0
ea
循环应力-应变曲线
a
K
)1 n
K为循环强度系数,应力量纲(MPa); n‟为循环应变硬化指数,无量纲。
弹性应变幅eea、塑性应变幅epa分别为:
a = Ee ea
a = K ' (e pa )
13
n
Cyclic stress-strain curve may be obtained from tests by using the samples method, in which a series of specimen are tested at various strain levels until the hysteresis loops become stabilized, than the stable hysteresis loops are superimposed and the tips of the loops are connected as shown in figure. 循环应力-应变曲线可用多试样法由 试验确定。这种方法是用一系列相 同试样在不同的应变水平下试验, 直到滞后环稳定,然后将这些稳态 环叠在一起,连接其顶点如图。
16
2. 变幅循环下的-e响应计算
已知变应变循环历程,取从最 大峰或谷起止的典型谱段,分 析
e
1 3 2 0 6 8 1'
5
2’ 7
5'
7'
t
e1 = ( 1 E ) + (1 K )
1 n
4
已知e1,用数值方法可解出1。 1-2 卸载。已知载荷反向的变程De1-2 , 求D1-2。
6
4.1 单调应力-应变响应 monotonic stress-strain response 1. Basic definitions:
A0 A
P d l
工程应力S: Engineering stress
工程应变e: Engineering strain
P S= A 0
D l l -l 0 e= = l0 l0
4-5 加载。已知De4-5 , 求D4-5, 得到:e5=e4+De4-5 ;
e
1 3 2 5 2’ 7 7' 6 4 8 5'
1'
0
t
5=4+D4-5。
5-6 卸载。已知De5-6 , 求D5-6。进而求得 e6、 6。 6-7 加载。已知De6-7 , 求D6-7。进而求得 e7、 7。 7-8 卸载。已知De7-8 ,求D7-8。可得:e8、8。
e ea
De Dee Dep D D 1n D D 1 n = + = + ( ) 或者 De = + 2 ( ) 2K E 2 2 2 2E 2K 同样,若用应变表示应力,则有:
D=EDee
和
D=2K‟(Dep /2)n'
15
4.3 材料的记忆特性与变幅循环响应计算
1. 材料的记忆特性
第四章 应变疲劳
4.1 单调应力-应变响应
4.2 滞后环和循环应力-应变响应
4.3 材料的记忆特性与变幅循环 响应计算 4.4 应变疲劳性能 4.5 缺口应变分析
返回主目录
1
第四章 应变疲劳
研究应变-寿命关系
应变疲劳或低周应变疲劳:
载荷水平高 (>ys),寿命短 (N<104)。
The strain-life method is based on the observation that in many components the response of the material in critical locations (notches) is strain or deformation dependent.
1'
0
t
4
2-3 加载。已知De2-3, 由滞后环曲线可求 D2-3。 对于加载,有:e3=e2+De2-3; 3=2+D2-3。 3-4 卸载。经过2‟处时,应变曾在该处 (2处)发生 过反向,由记忆特性知2-3-2‟形成封闭环, 且不影响其后的-e响应。
18
按路径 1-2-4计算-e响应,有: D 1- 4 D 1- 4 1 n D e 1- 4 = + 2( ) E 2K 得到: e4=e1-De1-4; 4=1-D1-4。
载荷水平低的时候,应力和应变是线性相关的。 因此,在这一范围内,应力控制和应变控制试验 的结果等效。在高载荷水平,即低周疲劳范围内, 循环应力应变响应和材料的性能在应变控制条件 下模拟更好。
3
Although most engineering structures and components are designed such that the nominal loads remain elastic, stress concentration may cause plastic strain to develop in the vicinity of notches. 尽管大部分工程结构和构件设计的名 义载荷是保持弹性的,应力集中也会在缺 口附近引起塑性应变。
9
2. monotonic stress-strain curve
均匀变形阶段,-e曲线上任一点 的应变e,均可表示为:
A
e=ee+ep
0
ep
ee
e
-ee关系用Hooke定理表达为:=Eee
