公司理财英文版第四章
英文版公司理财课件chapter 4

If you start investing now, time can be a very powerful ally. Year after year, the money you invest earns more money. And if you reinvest your earnings, you can earn even more money in the future, it’s called compounding returns and its one of the keys to making your money work harder.
13
For compounding to work its magic, you need to do two things:
1. Reinvest your investment returns (e.g. dividends and interest), rather than spending the money on other things. This will enable you to turn your investment earnings into capital so that you can generate even more future earnings. An easy way to reinvest income is to participate in a dividend, interest or income reinvestment scheme.
15
example
If you invest $10 000 at an 8% annual return until age 65, the table below shows how much you would get back
(完整版)公司理财部分课后答案

Chapter 1Goal OF FirmN0.2, 3, 4, 5, 10.2. Not-for-Profit Firm Goals Suppose you were the financial manager of a not-for-profit business (anot-for-profit hospital, perhaps). What kinds of goals do you think would be appropriate?答:所有者权益的市场价值的最大化。
3.Goal of the Firm Evaluate the following statement: Managers should not focus on the current stock value because doing so will lead to an overemphasis on short-term profits at the expense of long-term profits.答:错误;因为现在的股票价值已经反应了短期和长期的的风险、时间以及未来现金流量。
4. Ethics and Firm Goals Can the goal of maximizing the value of the stock conflict with other goals, such as avoiding unethical or illegal behavior? In particular, do you think subjects like customer and employee safety, the environment, and the general good of society fit in this framework, or are they essentially ignored? Think of some specific scenarios to illustrate your answer.答:有两种极端。
罗斯《公司理财》英文习题答案DOCchap004
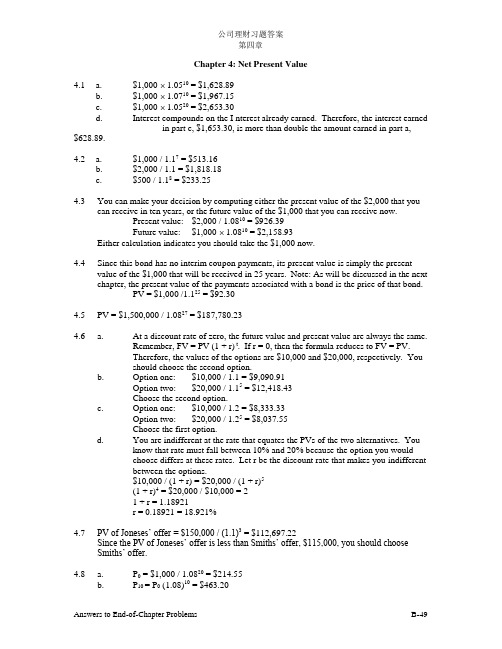
公司理财习题答案第四章Chapter 4: Net Present Value4.1 a. $1,000 ⨯ 1.0510 = $1,628.89b. $1,000 ⨯ 1.0710 = $1,967.15c. $1,000 ⨯ 1.0520 = $2,653.30d. Interest compounds on the I nterest already earned. Therefore, the interest earnedin part c, $1,653.30, is more than double the amount earned in part a, $628.89.4.2 a. $1,000 / 1.17 = $513.16b. $2,000 / 1.1 = $1,818.18c. $500 / 1.18 = $233.254.3 You can make your decision by computing either the present value of the $2,000 that youcan receive in ten years, or the future value of the $1,000 that you can receive now.Present value: $2,000 / 1.0810 = $926.39Future value: $1,000 ⨯ 1.0810 = $2,158.93Either calculation indicates you should take the $1,000 now.4.4 Since this bond has no interim coupon payments, its present value is simply the presentvalue of the $1,000 that will be received in 25 years. Note: As will be discussed in the next chapter, the present value of the payments associated with a bond is the price of that bond.PV = $1,000 /1.125 = $92.304.5 PV = $1,500,000 / 1.0827 = $187,780.234.6 a. At a discount rate of zero, the future value and present value are always the same.Remember, FV = PV (1 + r) t. If r = 0, then the formula reduces to FV = PV.Therefore, the values of the options are $10,000 and $20,000, respectively. Youshould choose the second option.b. Option one: $10,000 / 1.1 = $9,090.91Option two: $20,000 / 1.15 = $12,418.43Choose the second option.c. Option one: $10,000 / 1.2 = $8,333.33Option two: $20,000 / 1.25 = $8,037.55Choose the first option.d. You are indifferent at the rate that equates the PVs of the two alternatives. Youknow that rate must fall between 10% and 20% because the option you wouldchoose differs at these rates. Let r be the discount rate that makes you indifferentbetween the options.$10,000 / (1 + r) = $20,000 / (1 + r)5(1 + r)4 = $20,000 / $10,000 = 21 + r = 1.18921r = 0.18921 = 18.921%4.7 PV of Joneses’ offer = $150,000 / (1.1)3 = $112,697.22Since the PV of Joneses’ offer is less than Smiths’ offer, $115,000, you should chooseSmiths’ offer.4.8 a. P0 = $1,000 / 1.0820 = $214.55b. P10 = P0 (1.08)10 = $463.20c. P15 = P0 (1.08)15 = $680.594.9 The $1,000 that you place in the account at the end of the first year will earn interest for sixyears. The $1,000 that you place in the account at the end of the second year will earninterest for five years, etc. Thus, the account will have a balance of$1,000 (1.12)6 + $1,000 (1.12)5 + $1,000 (1.12)4 + $1,000 (1.12)3= $6,714.614.10 PV = $5,000,000 / 1.1210 = $1,609,866.184.11 a. The cost of investment is $900,000.PV of cash inflows = $120,000 / 1.12 + $250,000 / 1.122 + $800,000 / 1.123= $875,865.52Since the PV of cash inflows is less than the cost of investment, you should notmake the investment.b. NPV = -$900,000 + $875,865.52= -$24,134.48c. NPV = -$900,000 + $120,000 / 1.11 + $250,000 / 1.112 + $800,000 / 1.113= $-4,033.18Since the NPV is still negative, you should not make the investment.4.12 NPV = -($340,000 + $10,000) + ($100,000 - $10,000) / 1.1+ $90,000 / 1.12 + $90,000 / 1.13 + $90,000 / 1.14 + $100,000 / 1.15= -$2,619.98Since the NPV is negative, you should not buy it.If the relevant cost of capital is 9 percent,NPV = -$350,000 + $90,000 / 1.09 + $90,000 / 1.092 + $90,000 / 1.093+ $90,000 / 1.094 + $100,000 / 1.095= $6,567.93Since the NPV is positive, you should buy it.4.13 a. Profit = PV of revenue - Cost = NPVNPV = $90,000 / 1.15 - $60,000 = -$4,117.08No, the firm will not make a profit.b. Find r that makes zero NPV.$90,000 / (1+r)5 - $60,000 = $0(1+r)5 = 1.5r = 0.08447 = 8.447%4.14 The future value of the decision to own your car for one year is the sum of the trade-invalue and the benefit from owning the car. Therefore, the PV of the decision to own thecar for one year is$3,000 / 1.12 + $1,000 / 1.12 = $3,571.43Since the PV of the roommate’s offer, $3,500, is lower than the aunt’s offer, you shouldaccept aunt’s offer.4.15 a. $1.000 (1.08)3 = $1,259.71b. $1,000 [1 + (0.08 / 2)]2 ⨯ 3 = $1,000 (1.04)6 = $1,265.32c. $1,000 [1 + (0.08 / 12)]12 ⨯ 3 = $1,000 (1.00667)36 = $1,270.24d. $1,000 e0.08 ⨯ 3 = $1,271.25公司理财习题答案第四章e. The future value increases because of the compounding. The account is earninginterest on interest. Essentially, the interest is added to the account balance at theend of every compounding period. During the next period, the account earnsinterest on the new balance. When the compounding period shortens, the balancethat earns interest is rising faster.4.16 a. $1,000 e0.12 ⨯ 5 = $1,822.12b. $1,000 e0.1 ⨯ 3 = $1,349.86c. $1,000 e0.05 ⨯ 10 = $1,648.72d. $1,000 e0.07 ⨯ 8 = $1,750.674.17 PV = $5,000 / [1+ (0.1 / 4)]4 ⨯ 12 = $1,528.364.18 Effective annual interest rate of Bank America= [1 + (0.041 / 4)]4 - 1 = 0.0416 = 4.16%Effective annual interest rate of Bank USA= [1 + (0.0405 / 12)]12 - 1 = 0.0413 = 4.13%You should deposit your money in Bank America.4.19 The price of the consol bond is the present value of the coupon payments. Apply theperpetuity formula to find the present value. PV = $120 / 0.15 = $8004.20 Quarterly interest rate = 12% / 4 = 3% = 0.03Therefore, the price of the security = $10 / 0.03 = $333.334.21 The price at the end of 19 quarters (or 4.75 years) from today = $1 / (0.15 ÷ 4) = $26.67The current price = $26.67 / [1+ (.15 / 4)]19 = $13.254.22 a. $1,000 / 0.1 = $10,000b. $500 / 0.1 = $5,000 is the value one year from now of the perpetual stream. Thus,the value of the perpetuity is $5,000 / 1.1 = $4,545.45.c. $2,420 / 0.1 = $24,200 is the value two years from now of the perpetual stream.Thus, the value of the perpetuity is $24,200 / 1.12 = $20,000.4.23 The value at t = 8 is $120 / 0.1 = $1,200.Thus, the value at t = 5 is $1,200 / 1.13 = $901.58.4.24 P = $3 (1.05) / (0.12 - 0.05) = $45.004.25 P = $1 / (0.1 - 0.04) = $16.674.26 The first cash flow will be generated 2 years from today.The value at the end of 1 year from today = $200,000 / (0.1 - 0.05) = $4,000,000.Thus, PV = $4,000,000 / 1.1 = $3,636,363.64.4.27 A zero NPV-$100,000 + $50,000 / r = 0-r = 0.54.28 Apply the NPV technique. Since the inflows are an annuity you can use the present valueof an annuity factor.NPV = -$6,200 + $1,200 8A1.0= -$6,200 + $1,200 (5.3349)= $201.88Yes, you should buy the asset.4.29 Use an annuity factor to compute the value two years from today of the twenty payments.Remember, the annuity formula gives you the value of the stream one year before the first payment. Hence, the annuity factor will give you the value at the end of year two of the stream of payments. Value at the end of year two = $2,000 20A08.0= $2,000 (9.8181)= $19,636.20The present value is simply that amount discounted back two years.PV = $19,636.20 / 1.082 = $16,834.884.30 The value of annuity at the end of year five= $500 15A = $500 (5.84737) = $2,923.6915.0The present value = $2,923.69 / 1.125 = $1,658.984.31 The easiest way to do this problem is to use the annuity factor. The annuity factor must beequal to $12,800 / $2,000 = 6.4; remember PV =C A t r. The annuity factors are in theappendix to the text. To use the factor table to solve this problem, scan across the rowlabeled 10 years until you find 6.4. It is close to the factor for 9%, 6.4177. Thus, the rate you will receive on this note is slightly more than 9%.You can find a more precise answer by interpolating between nine and ten percent.10% ⎤ 6.1446 ⎤a ⎡ r ⎥bc ⎡ 6.4 ⎪ d⎣ 9% ⎦⎣ 6.4177 ⎦By interpolating, you are presuming that the ratio of a to b is equal to the ratio of c to d.(9 - r ) / (9 - 10) = (6.4177 - 6.4 ) / (6.4177 - 6.1446)r = 9.0648%The exact value could be obtained by solving the annuity formula for the interest rate.Sophisticated calculators can compute the rate directly as 9.0626%.公司理财习题答案第四章4.32 a. The annuity amount can be computed by first calculating the PV of the $25,000which you need in five years. That amount is $17,824.65 [= $25,000 / 1.075].Next compute the annuity which has the same present value.$17,824.65 = C 5A.007$17,824.65 = C (4.1002)C = $4,347.26Thus, putting $4,347.26 into the 7% account each year will provide $25,000 fiveyears from today.b. The lump sum payment must be the present value of the $25,000, i.e., $25,000 /1.075 = $17,824.65The formula for future value of any annuity can be used to solve the problem (seefootnote 14 of the text).4.33The amount of loan is $120,000 ⨯ 0.85 = $102,000.20C A= $102,000.010The amount of equal installments isC = $102,000 / 20A = $102,000 / 8.513564 = $11,980.8810.04.34 The present value of salary is $5,000 36A = $150,537.53.001The present value of bonus is $10,000 3A = $23,740.42 (EAR = 12.68% is used since.01268bonuses are paid annually.)The present value of the contract = $150,537.53 + $23,740.42 = $174,277.944.35 The amount of loan is $15,000 ⨯ 0.8 = $12,000.C 48A = $12,0000067.0The amount of monthly installments isC = $12,000 / 48A = $12,000 / 40.96191 = $292.960067.04.36 Option one: This cash flow is an annuity due. To value it, you must use the after-taxamounts. The after-tax payment is $160,000 (1 - 0.28) = $115,200. Value all except the first payment using the standard annuity formula, then add back the first payment of$115,200 to obtain the value of this option.Value = $115,200 + $115,200 30A10.0= $115,200 + $115,200 (9.4269)= $1,201,178.88Option two: This option is valued similarly. You are able to have $446,000 now; this is already on an after-tax basis. You will receive an annuity of $101,055 for each of the next thirty years. Those payments are taxable when you receive them, so your after-taxpayment is $72,759.60 [= $101,055 (1 - 0.28)].Value = $446,000 + $72,759.60 30A.010= $446,000 + $72,759.60 (9.4269)= $1,131,897.47Since option one has a higher PV, you should choose it.4.37 The amount of loan is $9,000. The monthly payment C is given by solving the equation: C 60008.0A = $9,000 C = $9,000 / 47.5042 = $189.46In October 2000, Susan Chao has 35 (= 12 ⨯ 5 - 25) monthly payments left, including the one due in October 2000.Therefore, the balance of the loan on November 1, 2000 = $189.46 + $189.46 34008.0A = $189.46 + $189.46 (29.6651) = $5,809.81Thus, the total amount of payoff = 1.01 ($5,809.81) = $5,867.91 4.38 Let r be the rate of interest you must earn. $10,000(1 + r)12 = $80,000 (1 + r)12 = 8 r = 0.18921 = 18.921%4.39 First compute the present value of all the payments you must make for your children’s education. The value as of one year before matriculation of one child’s education is$21,000 415.0A= $21,000 (2.8550) = $59,955. This is the value of the elder child’s education fourteen years from now. It is the value of the younger child’s education sixteen years from today. The present value of these is PV = $59,955 / 1.1514 + $59,955 / 1.1516 = $14,880.44You want to make fifteen equal payments into an account that yields 15% so that the present value of the equal payments is $14,880.44. Payment = $14,880.44 / 1515.0A = $14,880.44 / 5.8474 = $2,544.804.40 The NPV of the policy isNPV = -$750 306.0A - $800306.0A / 1.063 + $250,000 / [(1.066) (1.0759)] = -$2,004.76 - $1,795.45 + $3,254.33= -$545.88 Therefore, you should not buy the policy.4.41 The NPV of the lease offer isNPV = $120,000 - $15,000 - $15,000 908.0A - $25,000 / 1.0810= $105,000 - $93,703.32 - $11,579.84 = -$283.16 Therefore, you should not accept the offer.4.42 This problem applies the growing annuity formula. The first payment is $50,000(1.04)2(0.02) = $1,081.60. PV = $1,081.60 [1 / (0.08 - 0.04) - {1 / (0.08 - 0.04)}{1.04 / 1.08}40]= $21,064.28 This is the present value of the payments, so the value forty years from today is $21,064.28 (1.0840) = $457,611.46公司理财习题答案第四章4.43 Use the discount factors to discount the individual cash flows. Then compute the NPV ofthe project. Notice that the four $1,000 cash flows form an annuity. You can still use the factor tables to compute their PV. Essentially, they form cash flows that are a six year annuity less a two year annuity. Thus, the appropriate annuity factor to use with them is 2.6198 (= 4.3553 - 1.7355).Year Cash Flow Factor PV 1 $700 0.9091 $636.37 2 900 0.8264 743.76 3 1,000 ⎤ 4 1,000 ⎥ 2.6198 2,619.80 5 1,000 ⎥ 6 1,000 ⎦ 7 1,250 0.5132 641.50 8 1,375 0.4665 641.44 Total $5,282.87NPV = -$5,000 + $5,282.87 = $282.87 Purchase the machine.4.44 Weekly inflation rate = 0.039 / 52 = 0.00075 Weekly interest rate = 0.104 / 52 = 0.002 PV = $5 [1 / (0.002 - 0.00075)] {1 – [(1 + 0.00075) / (1 + 0.002)]52 ⨯ 30} = $3,429.384.45 Engineer:NPV = -$12,000 405.0A + $20,000 / 1.055 + $25,000 / 1.056 - $15,000 / 1.057- $15,000 / 1.058 + $40,000 2505.0A / 1.058= $352,533.35 Accountant:NPV = -$13,000 405.0A + $31,000 3005.0A / 1.054= $345,958.81 Become an engineer.After your brother announces that the appropriate discount rate is 6%, you can recalculate the NPVs. Calculate them the same way as above except using the 6% discount rate. Engineer NPV = $292,419.47 Accountant NPV = $292,947.04Your brother made a poor decision. At a 6% rate, he should study accounting.4.46 Since Goose receives his first payment on July 1 and all payments in one year intervalsfrom July 1, the easiest approach to this problem is to discount the cash flows to July 1 then use the six month discount rate (0.044) to discount them the additional six months. PV = $875,000 / (1.044) + $650,000 / (1.044)(1.09) + $800,000 / (1.044)(1.092) + $1,000,000 / (1.044)(1.093) + $1,000,000/(1.044)(1.094) + $300,000 / (1.044)(1.095)+ $240,000 1709.0A / (1.044)(1.095) + $125,000 1009.0A / (1.044)(1.0922) = $5,051,150Remember that the use of annuity factors to discount the deferred payments yields the value of the annuity stream one period prior to the first payment. Thus, the annuity factor applied to the first set of deferred payments gives the value of those payments on July 1 of 1989. Discounting by 9% for five years brings the value to July 1, 1984. The use of the six month discount rate (4.4%) brings the value of the payments to January 1, 1984. Similarly, the annuity factor applied to the second set of deferred payments yields the value of those payments in 2006. Discounting for 22 years at 9% and for six months at 4.4% provides the value at January 1, 1984.The equivalent five-year, annual salary is the annuity that solves: $5,051,150 = C 509.0A C = $5,051,150/3.8897C = $1,298,596The student must be aware of possible rounding errors in this problem. The differencebetween 4.4% semiannual and 9.0% and for six months at 4.4% provides the value at January 1, 1984. 4.47 PV = $10,000 + ($35,000 + $3,500) [1 / (0.12 - 0.04)] [1 - (1.04 / 1.12) 25 ]= $415,783.604.48 NPV = -$40,000 + $10,000 [1 / (0.10 - 0.07)] [1 - (1.07 / 1.10)5 ] = $3,041.91 Revise the textbook.4.49The amount of the loan is $400,000 (0.8) = $320,000 The monthly payment is C = $320,000 / 3600067.0.0A = $ 2,348.10 Thirty years of payments $ 2,348.10 (360) = $ 845,316.00 Eight years of payments $2,348.10 (96) = $225,417.60 The difference is the balloon payment of $619,898.404.50 The lease payment is an annuity in advanceC + C 2301.0A = $4,000 C (1 + 20.4558) = $4,000 C = $186.424.51 The effective annual interest rate is[ 1 + (0.08 / 4) ] 4 – 1 = 0.0824The present value of the ten-year annuity is PV = 900 100824.0A = $5,974.24 Four remaining discount periodsPV = $5,974.24 / (1.0824) 4 = $4,352.43公司理财习题答案第四章4.52The present value of Ernie’s retirement incomePV = $300,000 20A / (1.07) 30 = $417,511.5407.0The present value of the cabinPV = $350,000 / (1.07) 10 = $177,922.25The present value of his savingsPV = $40,000 10A = $280,943.26.007In present value terms he must save an additional $313,490.53 In future value termsFV = $313,490.53 (1.07) 10 = $616,683.32He must saveC = $616.683.32 / 20A = $58,210.5407.0。
公司理财原版英文课件Chap024

24-5
Dilution Example
Firm s value net of debt ' − exercise price # The gain from exercising a warrant can be written as: Firm s value net of debt + exercise price×#w ' − exercise price #+#w
24-6
Dilution Example
Suppose that Mr. Armstrong and Mr. LeMond meet as the board of directors of LegStrong. The board decides to sell Mr. Mercx a warrant. The warrant gives Mr. Mercx the option to buy one share for $1,500. Suppose the warrant finishes in-the-money, (gold increased to $350 per ounce). Mr. Mercx will exercise. The firm will print up one new share.
Chapter 24
Warrants and Convertibles
McGraw-Hill/Irwin
罗斯《公司理财》第9版英文原书课后部分章节答案

罗斯《公司理财》第9版精要版英文原书课后部分章节答案详细»1 / 17 CH5 11,13,18,19,20 11. To find the PV of a lump sum, we use: PV = FV / (1 + r) t PV = $1,000,000 / (1.10) 80 = $488.19 13. To answer this question, we can use either the FV or the PV formula. Both will give the same answer since they are the inverse of each other. We will use the FV formula, that is: FV = PV(1 + r) t Solving for r, we get: r = (FV / PV) 1 / t –1 r = ($1,260,000 / $150) 1/112 – 1 = .0840 or 8.40% To find the FV of the first prize, we use: FV = PV(1 + r) t FV = $1,260,000(1.0840) 33 = $18,056,409.94 18. To find the FV of a lump sum, we use: FV = PV(1 + r) t FV = $4,000(1.11) 45 = $438,120.97 FV = $4,000(1.11) 35 = $154,299.40 Better start early! 19. We need to find the FV of a lump sum. However, the money will only be invested for six years, so the number of periods is six. FV = PV(1 + r) t FV = $20,000(1.084)6 = $32,449.33 20. To answer this question, we can use either the FV or the PV formula. Both will give the same answer since they are the inverse of each other. We will use the FV formula, that is: FV = PV(1 + r) t Solving for t, we get: t = ln(FV / PV) / ln(1 + r) t = ln($75,000 / $10,000) / ln(1.11) = 19.31 So, the money must be invested for 19.31 years. However, you will not receive the money for another two years. From now, you’ll wait: 2 years + 19.31 years = 21.31 years CH6 16,24,27,42,58 16. For this problem, we simply need to find the FV of a lump sum using the equation: FV = PV(1 + r) t 2 / 17 It is important to note that compounding occurs semiannually. To account for this, we will divide the interest rate by two (the number of compounding periods in a year), and multiply the number of periods by two. Doing so, we get: FV = $2,100[1 + (.084/2)] 34 = $8,505.93 24. This problem requires us to find the FV A. The equation to find the FV A is: FV A = C{[(1 + r) t – 1] / r} FV A = $300[{[1 + (.10/12) ] 360 – 1} / (.10/12)] = $678,146.38 27. The cash flows are annual and the compounding period is quarterly, so we need to calculate the EAR to make the interest rate comparable with the timing of the cash flows. Using the equation for the EAR, we get: EAR = [1 + (APR / m)] m – 1 EAR = [1 + (.11/4)] 4 – 1 = .1146 or 11.46% And now we use the EAR to find the PV of each cash flow as a lump sum and add them together: PV = $725 / 1.1146 + $980 / 1.1146 2 + $1,360 / 1.1146 4 = $2,320.36 42. The amount of principal paid on the loan is the PV of the monthly payments you make. So, the present value of the $1,150 monthly payments is: PV A = $1,150[(1 – {1 / [1 + (.0635/12)]} 360 ) / (.0635/12)] = $184,817.42 The monthly payments of $1,150 will amount to a principal payment of $184,817.42. The amount of principal you will still owe is: $240,000 – 184,817.42 = $55,182.58 This remaining principal amount will increase at the interest rate on the loan until the end of the loan period. So the balloon payment in 30 years, which is the FV of the remaining principal will be: Balloon payment = $55,182.58[1 + (.0635/12)] 360 = $368,936.54 58. To answer this question, we should find the PV of both options, and compare them. Since we are purchasing the car, the lowest PV is the best option. The PV of the leasing is simply the PV of the lease payments, plus the $99. The interest rate we would use for the leasing option is the same as the interest rate of the loan. The PV of leasing is: PV = $99 + $450{1 –[1 / (1 + .07/12) 12(3) ]} / (.07/12) = $14,672.91 The PV of purchasing the car is the current price of the car minus the PV of the resale price. The PV of the resale price is: PV = $23,000 / [1 + (.07/12)] 12(3) = $18,654.82 The PV of the decision to purchase is: $32,000 – 18,654.82 = $13,345.18 3 / 17 In this case, it is cheaper to buy the car than leasing it since the PV of the purchase cash flows is lower. To find the breakeven resale price, we need to find the resale price that makes the PV of the two options the same. In other words, the PV of the decision to buy should be: $32,000 – PV of resale price = $14,672.91 PV of resale price = $17,327.09 The resale price that would make the PV of the lease versus buy decision is the FV ofthis value, so: Breakeven resale price = $17,327.09[1 + (.07/12)] 12(3) = $21,363.01 CH7 3,18,21,22,31 3. The price of any bond is the PV of the interest payment, plus the PV of the par value. Notice this problem assumes an annual coupon. The price of the bond will be: P = $75({1 – [1/(1 + .0875)] 10 } / .0875) + $1,000[1 / (1 + .0875) 10 ] = $918.89 We would like to introduce shorthand notation here. Rather than write (or type, as the case may be) the entire equation for the PV of a lump sum, or the PV A equation, it is common to abbreviate the equations as: PVIF R,t = 1 / (1 + r) t which stands for Present V alue Interest Factor PVIFA R,t = ({1 – [1/(1 + r)] t } / r ) which stands for Present V alue Interest Factor of an Annuity These abbreviations are short hand notation for the equations in which the interest rate and the number of periods are substituted into the equation and solved. We will use this shorthand notation in remainder of the solutions key. 18. The bond price equation for this bond is: P 0 = $1,068 = $46(PVIFA R%,18 ) + $1,000(PVIF R%,18 ) Using a spreadsheet, financial calculator, or trial and error we find: R = 4.06% This is thesemiannual interest rate, so the YTM is: YTM = 2 4.06% = 8.12% The current yield is:Current yield = Annual coupon payment / Price = $92 / $1,068 = .0861 or 8.61% The effective annual yield is the same as the EAR, so using the EAR equation from the previous chapter: Effective annual yield = (1 + 0.0406) 2 – 1 = .0829 or 8.29% 20. Accrued interest is the coupon payment for the period times the fraction of the period that has passed since the last coupon payment. Since we have a semiannual coupon bond, the coupon payment per six months is one-half of the annual coupon payment. There are four months until the next coupon payment, so two months have passed since the last coupon payment. The accrued interest for the bond is: Accrued interest = $74/2 × 2/6 = $12.33 And we calculate the clean price as: 4 / 17 Clean price = Dirty price –Accrued interest = $968 –12.33 = $955.67 21. Accrued interest is the coupon payment for the period times the fraction of the period that has passed since the last coupon payment. Since we have a semiannual coupon bond, the coupon payment per six months is one-half of the annual coupon payment. There are two months until the next coupon payment, so four months have passed since the last coupon payment. The accrued interest for the bond is: Accrued interest = $68/2 × 4/6 = $22.67 And we calculate the dirty price as: Dirty price = Clean price + Accrued interest = $1,073 + 22.67 = $1,095.67 22. To find the number of years to maturity for the bond, we need to find the price of the bond. Since we already have the coupon rate, we can use the bond price equation, and solve for the number of years to maturity. We are given the current yield of the bond, so we can calculate the price as: Current yield = .0755 = $80/P 0 P 0 = $80/.0755 = $1,059.60 Now that we have the price of the bond, the bond price equation is: P = $1,059.60 = $80[(1 – (1/1.072) t ) / .072 ] + $1,000/1.072 t We can solve this equation for t as follows: $1,059.60(1.072) t = $1,111.11(1.072) t –1,111.11 + 1,000 111.11 = 51.51(1.072) t2.1570 = 1.072 t t = log 2.1570 / log 1.072 = 11.06 11 years The bond has 11 years to maturity.31. The price of any bond (or financial instrument) is the PV of the future cash flows. Even though Bond M makes different coupons payments, to find the price of the bond, we just find the PV of the cash flows. The PV of the cash flows for Bond M is: P M = $1,100(PVIFA 3.5%,16 )(PVIF 3.5%,12 ) + $1,400(PVIFA3.5%,12 )(PVIF 3.5%,28 ) + $20,000(PVIF 3.5%,40 ) P M = $19,018.78 Notice that for the coupon payments of $1,400, we found the PV A for the coupon payments, and then discounted the lump sum back to today. Bond N is a zero coupon bond with a $20,000 par value, therefore, the price of the bond is the PV of the par, or: P N = $20,000(PVIF3.5%,40 ) = $5,051.45 CH8 4,18,20,22,244. Using the constant growth model, we find the price of the stock today is: P 0 = D 1 / (R – g) = $3.04 / (.11 – .038) = $42.22 5 / 17 18. The price of a share of preferred stock is the dividend payment divided by the required return. We know the dividend payment in Year 20, so we can find the price of the stock in Y ear 19, one year before the first dividend payment. Doing so, we get: P 19 = $20.00 / .064 P 19 = $312.50 The price of the stock today is the PV of the stock price in the future, so the price today will be: P 0 = $312.50 / (1.064) 19 P 0 = $96.15 20. We can use the two-stage dividend growth model for this problem, which is: P 0 = [D 0 (1 + g 1 )/(R – g 1 )]{1 – [(1 + g 1 )/(1 + R)] T }+ [(1 + g 1 )/(1 + R)] T [D 0 (1 + g 2 )/(R –g 2 )] P0 = [$1.25(1.28)/(.13 –.28)][1 –(1.28/1.13) 8 ] + [(1.28)/(1.13)] 8 [$1.25(1.06)/(.13 – .06)] P 0 = $69.55 22. We are asked to find the dividend yield and capital gains yield for each of the stocks. All of the stocks have a 15 percent required return, which is the sum of the dividend yield and the capital gains yield. To find the components of the total return, we need to find the stock price for each stock. Using this stock price and the dividend, we can calculate the dividend yield. The capital gains yield for the stock will be the total return (required return) minus the dividend yield. W: P 0 = D 0 (1 + g) / (R – g) = $4.50(1.10)/(.19 – .10) = $55.00 Dividend yield = D 1 /P 0 = $4.50(1.10)/$55.00 = .09 or 9% Capital gains yield = .19 – .09 = .10 or 10% X: P 0 = D 0 (1 + g) / (R – g) = $4.50/(.19 – 0) = $23.68 Dividend yield = D 1 /P 0 = $4.50/$23.68 = .19 or 19% Capital gains yield = .19 – .19 = 0% Y: P 0 = D 0 (1 + g) / (R – g) = $4.50(1 – .05)/(.19 + .05) = $17.81 Dividend yield = D 1 /P 0 = $4.50(0.95)/$17.81 = .24 or 24% Capital gains yield = .19 – .24 = –.05 or –5% Z: P 2 = D 2 (1 + g) / (R – g) = D 0 (1 + g 1 ) 2 (1 +g 2 )/(R – g 2 ) = $4.50(1.20) 2 (1.12)/(.19 – .12) = $103.68 P 0 = $4.50 (1.20) / (1.19) + $4.50(1.20) 2 / (1.19) 2 + $103.68 / (1.19) 2 = $82.33 Dividend yield = D 1 /P 0 = $4.50(1.20)/$82.33 = .066 or 6.6% Capital gains yield = .19 – .066 = .124 or 12.4% In all cases, the required return is 19%, but the return is distributed differently between current income and capital gains. High growth stocks have an appreciable capital gains component but a relatively small current income yield; conversely, mature, negative-growth stocks provide a high current income but also price depreciation over time. 24. Here we have a stock with supernormal growth, but the dividend growth changes every year for the first four years. We can find the price of the stock in Y ear 3 since the dividend growth rate is constant after the third dividend. The price of the stock in Y ear 3 will be the dividend in Y ear 4, divided by the required return minus the constant dividend growth rate. So, the price in Y ear 3 will be: 6 / 17 P3 = $2.45(1.20)(1.15)(1.10)(1.05) / (.11 – .05) = $65.08 The price of the stock today will be the PV of the first three dividends, plus the PV of the stock price in Y ear 3, so: P 0 = $2.45(1.20)/(1.11) + $2.45(1.20)(1.15)/1.11 2 + $2.45(1.20)(1.15)(1.10)/1.11 3 + $65.08/1.11 3 P 0 = $55.70 CH9 3,4,6,9,15 3. Project A has cash flows of $19,000 in Y ear 1, so the cash flows are short by $21,000 of recapturing the initial investment, so the payback for Project A is: Payback = 1 + ($21,000 / $25,000) = 1.84 years Project B has cash flows of: Cash flows = $14,000 + 17,000 + 24,000 = $55,000 during this first three years. The cash flows are still short by $5,000 of recapturing the initial investment, so the payback for Project B is: B: Payback = 3 + ($5,000 / $270,000) = 3.019 years Using the payback criterion and a cutoff of 3 years, accept project A and reject project B. 4. When we use discounted payback, we need to find the value of all cash flows today. The value today of the project cash flows for the first four years is: V alue today of Y ear 1 cash flow = $4,200/1.14 = $3,684.21 V alue today of Y ear 2 cash flow = $5,300/1.14 2 = $4,078.18 V alue today of Y ear 3 cash flow = $6,100/1.14 3 = $4,117.33 V alue today of Y ear 4 cash flow = $7,400/1.14 4 = $4,381.39 To findthe discounted payback, we use these values to find the payback period. The discounted first year cash flow is $3,684.21, so the discounted payback for a $7,000 initial cost is: Discounted payback = 1 + ($7,000 – 3,684.21)/$4,078.18 = 1.81 years For an initial cost of $10,000, the discounted payback is: Discounted payback = 2 + ($10,000 –3,684.21 –4,078.18)/$4,117.33 = 2.54 years Notice the calculation of discounted payback. We know the payback period is between two and three years, so we subtract the discounted values of the Y ear 1 and Y ear 2 cash flows from the initial cost. This is the numerator, which is the discounted amount we still need to make to recover our initial investment. We divide this amount by the discounted amount we will earn in Y ear 3 to get the fractional portion of the discounted payback. If the initial cost is $13,000, the discounted payback is: Discounted payback = 3 + ($13,000 – 3,684.21 – 4,078.18 – 4,117.33) / $4,381.39 = 3.26 years 7 / 17 6. Our definition of AAR is the average net income divided by the average book value. The average net income for this project is: A verage net income = ($1,938,200 + 2,201,600 + 1,876,000 + 1,329,500) / 4 = $1,836,325 And the average book value is: A verage book value = ($15,000,000 + 0) / 2 = $7,500,000 So, the AAR for this project is: AAR = A verage net income / A verage book value = $1,836,325 / $7,500,000 = .2448 or 24.48% 9. The NPV of a project is the PV of the outflows minus the PV of the inflows. Since the cash inflows are an annuity, the equation for the NPV of this project at an 8 percent required return is: NPV = –$138,000 + $28,500(PVIFA 8%, 9 ) = $40,036.31 At an 8 percent required return, the NPV is positive, so we would accept the project. The equation for the NPV of the project at a 20 percent required return is: NPV = –$138,000 + $28,500(PVIFA 20%, 9 ) = –$23,117.45 At a 20 percent required return, the NPV is negative, so we would reject the project. We would be indifferent to the project if the required return was equal to the IRR of the project, since at that required return the NPV is zero. The IRR of the project is: 0 = –$138,000 + $28,500(PVIFA IRR, 9 ) IRR = 14.59% 15. The profitability index is defined as the PV of the cash inflows divided by the PV of the cash outflows. The equation for the profitability index at a required return of 10 percent is: PI = [$7,300/1.1 + $6,900/1.1 2 + $5,700/1.1 3 ] / $14,000 = 1.187 The equation for the profitability index at a required return of 15 percent is: PI = [$7,300/1.15 + $6,900/1.15 2 + $5,700/1.15 3 ] / $14,000 = 1.094 The equation for the profitability index at a required return of 22 percent is: PI = [$7,300/1.22 + $6,900/1.22 2 + $5,700/1.22 3 ] / $14,000 = 0.983 8 / 17 We would accept the project if the required return were 10 percent or 15 percent since the PI is greater than one. We would reject the project if the required return were 22 percent since the PI。
公司理财第四章筹资成本分析-精品

32
EBIT/ EBIT DOL= x/ x
= EBIT/ EBIT (px)/ px
式中: DOL为经营杠杆系 数; EBIT为变动前的息 税前利润; ΔEBIT为息税前利 润的变动额; px为变动前的销售 收入; Δ(px)为销售收入 的变动额; x为变动前的产销量; Δx为产销量的变动 数。
2020/5/29
西南政法大学
24
(1)固定成本
固定成本,是指其总额在一定时期和一 定业务量范围内不随业务量发生任何变 动的那部分成本。
属于固定成本的主要有按直线法计提 的折旧费、保险费、管理人员工资、办 公费等,这些费用每年支出水平基本相 同,即使产销业务量在一定范围内变动, 它们也保持固定不变。
p为销售单价; b为单位变动成本; x为产销量; m为单位边际贡献。
2020/5/29
西南政法大学
29
(三)息税前利润及其计算
息税前利润是指企业支付利息和交纳所得税 之前的利润。成本按习性分类后,息税前利润可 用下列公式计算:
EBIT=px-bx-a=(p-b)x-a=M-a
式中:EBIT为息税前利润;a为固定成本。
2020/5/29
西南政法大学
25
(2)变动成本
变动成本是指其总额随着业务量成 正比例变动的那部分成本。
直接材料、直接人工等都属于变动 成本。
但从产品的单位成本来看,则恰好 相反,产品单位成本中的直接材料、直 接人工将保持不变。
2020/5/29
西南政法大学
26
(3)混合成本
有些成本虽然也随业务量的变动而变 动,但不成同比例变动,不能简单地归 人变动成本或固定成本,这类成本称为 混合成本。
无风险利率Rf一般用同期国库券收益率表示, 这是证券市场最基础的数据。
(完整版)公司理财-罗斯课后习题答案

(完整版)公司理财-罗斯课后习题答案-CAL-FENGHAI-(2020YEAR-YICAI)_JINGBIAN第一章1.在所有权形式的公司中,股东是公司的所有者。
股东选举公司的董事会,董事会任命该公司的管理层。
企业的所有权和控制权分离的组织形式是导致的代理关系存在的主要原因。
管理者可能追求自身或别人的利益最大化,而不是股东的利益最大化。
在这种环境下,他们可能因为目标不一致而存在代理问题。
2.非营利公司经常追求社会或政治任务等各种目标。
非营利公司财务管理的目标是获取并有效使用资金以最大限度地实现组织的社会使命。
3.这句话是不正确的。
管理者实施财务管理的目标就是最大化现有股票的每股价值,当前的股票价值反映了短期和长期的风险、时间以及未来现金流量。
4.有两种结论。
一种极端,在市场经济中所有的东西都被定价。
因此所有目标都有一个最优水平,包括避免不道德或非法的行为,股票价值最大化。
另一种极端,我们可以认为这是非经济现象,最好的处理方式是通过政治手段。
一个经典的思考问题给出了这种争论的答案:公司估计提高某种产品安全性的成本是30美元万。
然而,该公司认为提高产品的安全性只会节省20美元万。
请问公司应该怎么做呢?”5.财务管理的目标都是相同的,但实现目标的最好方式可能是不同的,因为不同的国家有不同的社会、政治环境和经济制度。
6.管理层的目标是最大化股东现有股票的每股价值。
如果管理层认为能提高公司利润,使股价超过35美元,那么他们应该展开对恶意收购的斗争。
如果管理层认为该投标人或其它未知的投标人将支付超过每股35美元的价格收购公司,那么他们也应该展开斗争。
然而,如果管理层不能增加企业的价值,并且没有其他更高的投标价格,那么管理层不是在为股东的最大化权益行事。
现在的管理层经常在公司面临这些恶意收购的情况时迷失自己的方向。
7.其他国家的代理问题并不严重,主要取决于其他国家的私人投资者占比重较小。
较少的私人投资者能减少不同的企业目标。
《公司理财》斯蒂芬A.罗斯..,机械工业出版社 英文课件

McGraw-Hill/Irwin
Copyright © 2004 by The McGraw-Hill Companies, Inc. All rights reserved.
5-7
Pure Discount Bonds
Information needed for valuing pure discount bonds:
5-6
5.2 How to Value Bonds
• Identify the size and timing of cash flows. • Discount at the correct discount rate.
– If you know the price of a bond and the size and timing of cash flows, the yield to maturity is the discount rate.
– Time to maturity (T) = Maturity date - today’s date – Face value (F) – Discount rate (r)
$0
0
$0
$0
T 1
$F
T
1
2
Present value of a pure discount bond at time 0:
• To value bonds and stocks we need to:
– Estimate future cash flows:
• Size (how much)ຫໍສະໝຸດ and • Timing (when)
– Discount future cash flows at an appropriate rate:
公司理财学原理第4章习题答案

A.实际收益率加通货膨胀率
B.实际收益率减通货膨胀率
C.实际收益率乘通货膨胀率
D.实际收益率加通货膨胀率加上实际收益率乘通货膨胀率
13.当无风险收益率下降时,市场全部收益率线将会(B)。
A.向上移动
B.向下移动
C.向右平移
D.向左平移
三、多选题
1.年金是指一定时期内每期等额收付的系列款项,下列各项中属于年金形式的是(A B C D)。
B.3060.4
C.
【答案解析】注意本题是单利计息的情况,所以并不是求即付年金终值的问题,单利终值=1000×(1+3×2%)+1000×(1+2×2%)+1000×(1+2%)=3120(元)。
10.已知利率为10%的一期、两期、三期的复利现值系数分别是、、,则可以判断利率为10%,3年期的年金现值系数为(B)。
B.1104.1
C.1204
【答案解析】五年后可以取出的数额即存款的本利和=1000×(F/P,2%,5)=(元)。
3.某人进行一项投资,预计6年后会获得收益880元,在年利率为5%的情况下,这笔收益的现值为(B)元。
B.656.66
C.
【答案解析】收益的现值=880×(P/F,5%,6)=(元)。
8.某人拟进行一项投资,希望进行该项投资后每半年都可以获得1000元的收入,年收益率为10%,则目前的投资额应是(C)元。
.11000
C
【答案解析】本题是永续年金求现值的问题,注意是每半年可以获得1000元,所以折现率应当使用半年的收益率即5%,所以投资额=1000/5%=20000(元)。
9.某人在第一年、第二年、第三年年初分别存入1000元,年利率2%,单利计息的情况下,在第三年年末此人可以取出(A)元。
《corporate finance》罗斯版 英文版 Chapter 04书本课后习题及答案

Chapter 041.An annuity stream of cash flow payments is a set of:A. e qual cash flows occurring each time period over a fixed length of time.B. e qual cash flows occurring each time period forever.C. e ither equal or varying cash flows occurring at set intervals of time for a fixed period.D. i ncreasing cash flows occurring at set intervals of time that go on forever.E. a rbitrary cash flows occurring each time period for no more than 10 years.2.Annuities where the payments occur at the end of each time period are called _____, whereas_____ refer to annuity streams with payments occurring at the beginning of each time period.A. o rdinary annuities; early annuitiesB. l ate annuities; straight annuitiesC. s traight annuities; late annuitiesD. a nnuities due; ordinary annuitiesE. o rdinary annuities; annuities due3. A flow of unending and equal payments that occur at regular intervals of time is called a(n):A. a nnuity due.B. i ndemnity.C. p erpetuity.D. a mortized cash flow stream.E. a mortization table.4.An interest rate that is compounded monthly, but is expressed as if the rate were compoundedannually, is called the _____ rate.A. s tated interestB. c ompound interestC. e ffective annualD. p eriodic interestE. d aily interest5.The interest rate charged per period multiplied by the number of periods per year is called the_____ rate.A. e ffective annualB. a nnual percentageC. p eriodic interestD. c ompound interestE. d aily interest6.Binder and Sons borrowed $138,000 for three years from their local bank and now they arepaying monthly payments that include both principal and interest. Paying off debt by making installments payments, such as Binder and Sons is doing, is referred to as:A. f oreclosing on the debt.B. a mortizing the debt.C. f unding the debt.D. c alling the debt.E. r efunding the debt.7.Ted purchased an annuity today that will pay $1,000 a month for five years. He received his firstmonthly payment today. Allison purchased an annuity today that will pay $1,000 a month for five years. She will receive her first payment one month from today. Which one of the followingstatements is correct concerning these two annuities?A. B oth annuities are of equal value today.B. A llison’s annuity is an annuity due.C. T ed’s annuity has a higher present value than Allison’s.D. A llison’s annuity has a higher present value than Ted’s.E. T ed’s annuity is an ordinary annuity.8.You are comparing two investment options, each of which will provide $15,000 of total income.Option A pays five annual payments starting with $5,000 the first year followed by four annual payments of $2,500 each. Option B pays five annual payments of $3,000 each. Which one of the following statements is correct given these two investment options?A. B oth options are of equal value today.B. G iven a positive rate of return, Option A is worth more today than Option B.C. O ption B has a higher present value than Option A given a positive rate of return.D. O ption B has a lower present value than Option A given a zero rate of return.E. O ption A is preferable because it is an annuity due.9.You are considering two projects with the following cash flows:Assuming both projects have the same initial cost, you know that:A. t here are no conditions under which the projects can have equal values.B. P roject B has a higher net present value than Project A.C.Project A is more valuable than Project B given a positive discount rate.D.both projects offer the same rate of return.E.both projects have equal net present values at any discount rate.10.A perpetuity differs from an annuity because:A. p erpetuity payments vary with the rate of inflation.B. p erpetuity payments vary with the market rate of interest.C. p erpetuity payments are variable while annuity payments are constant.D. p erpetuity payments never cease.E. a nnuity payments occur at irregular intervals of time.11.The annual percentage rate:A. c onsiders interest on interest.B. i s the actual cost of a loan with monthly payments.C. i s higher than the effective annual rate when interest is compounded quarterly.D. i s the interest rate charged per period divided by (1 + n), when n is the number of periods peryear.E. e quals the effective annual rate when the interest on an account is designated as simpleinterest.12.You would be making a wise decision if you chose to:A. b ase decisions regarding investments on effective rates and base decisions regarding loanson annual percentage rates.B. a ssume all loans and investments are based on simple interest.C. a ccept the loan with the lower effective annual rate rather than the loan with the lower annualpercentage rate.D. i nvest in an account paying 6 percent, compounded quarterly, rather than an account paying 6percent, compounded monthly.E. i gnore the effective rates and concentrate on the annual percentage rates for all transactions.13.The highest effective annual rate that can be derived from an annual percentage rate of 9% iscomputed as:A. [1 + (.09 / 365)] × 365.B. e.09×q.C. e × (1 + .09).D. e.09−1.E. [1 + (.09 / 365)]365−1.14.Given a stated interest rate, which form of compounding will yield the highest effective rate ofinterest?A. a nnual compoundingB. m onthly compoundingC. d aily compoundingD. c ontinuous compoundingE. s emiannual compounding15.The net present value of a project is equal to the:A. p resent value of the future cash flows.B. p resent value of the future cash flows minus the initial cost.C. f uture value of the future cash flows minus the initial cost.D. f uture value of the future cash flows minus the present value of the initial cost.E. s um of the project’s anticipated cash flows.16.What is the present value of $6,811 to be received in one year if the discount rate is 6.5 percent?A.$6,395.31B.$6,023.58C.$6,643.29D.$6,671.13E.$7,253.7217.You plan to invest $6,500 for three years at 4 percent simple interest. What will your investmentbe worth at the end of the three years? A. $6,941.11A. A NSB. $7,280.00B. $7,311.62C. $7,250.00D. $6,760.0018.Shawn has $2,500 invested at a guaranteed rate of 4.35 percent, compounded annually. Whatwill his investment be worth after five years?A. $2,997.04B. $3,288.00C. $3,321.32D. $3,093.16E. $2,857.5919.Your parents plan to give you $200 a month for four years while you are in college. At a discountrate of 6 percent, compounded monthly, what are these payments worth to you when you first start college?A. $8,797.40B. $8,409.56C. $8,198.79D. $8,516.06E. $8,279.3220.You just won the lottery! As your prize you will receive $1,500 a month for 150 months. If you canearn 7 percent, compounded monthly, on your money, what is this prize worth to you today?A. $137,003.69B. $149,676.91C. $137,962.77D. $148,104.26E. $150,723.7621.Olivia is willing to pay $185 a month for four years for a car payment. If the interest rate is 4.9percent, compounded monthly, and she has a cash down payment of $2,500, what price car can she afford to purchase?A. $10,961.36B. $10,549.07C. $8,533.84D. $8,686.82E. $8,342.0522.You are the beneficiary of a life insurance policy. The insurance company offers two options forreceiving the proceeds: a lump sum of $50,000 today or payments of $550 a month for ten years.If you can earn 6 percent, compounded monthly, which option should you take and why?A. Y ou should accept the lump sum because the payments are only worth $49,540.40 today.B. Y ou should accept the payments because they are worth $51,523.74 today.C. Y ou should accept the payments because they are worth $53,737.08 today.D. Y ou should accept the $50,000 because the payments are only worth $49,757.69 today.E. Y ou should accept the $50,000 because the payments are only worth $48,808.17 today.23.Your employer contributes $50 a week to your retirement plan. Assume you work for youremployer for another twenty years and the applicable discount rate is 5 percent, compounded weekly. Given these assumptions, what is this employee benefit worth to you today?A. $29,144.43B. $35,920.55C. $32,861.08D. $26,446.34E. $36,519.0224.Wilt has a consulting contract with a firm that states that he will receive annual payments of$50,000 a year for five years with the first payment due today. What is the current value of this contract if the discount rate is 8.4 percent?A. $214,142.50B. $201,867.47C. $195,618.19D. $197,548.43E. $224,267.1025.Uptown Industries just decided to save $3,000 a quarter for the next three years. The money willearn 2.75 percent, compounded quarterly, and the first deposit will be made today. If thecompany had wanted to deposit one lump sum today, rather than make quarterly deposits, how much would it have had to deposit today to have the same amount saved at the end of the three years?A. $34,441.56B. $34,678.35C. $33,428.87D. $33,687.23E. $34,998.0126.You need some money today and the only friend you have that has any is your ‘miserly' friend.He agrees to loan you the money you need, if you make payments of $20 a month for the next six months. In keeping with his reputation, he requires that the first payment be paid today. He also charges you 1.5% interest per month. How much total interest does he expect to earn?A. $3.94B. $4.35C. $1.34D. $3.63E. $5.9627.Lois is purchasing an annuity that will pay $5,000 annually for 20 years, with the first annuitypayment made on the date of purchase. What is the value of the annuity on the purchase date given a discount rate of 7 percent?A. $54,282.98B. $52,970.07C. $56,677.98D. $56,191.91E. $66,916.2128.Denise will receive annual payments of $10,000 for the next 25 years. The discount rate is 6.8percent. What is the difference in the present value of these payments if they are paid at the beginning of each year rather than at the end of each year?A. $8,069B. $9,217C. $9,706D. $8,382E. $8,85029.You are comparing two annuities with equal present values. The applicable discount rate is 6.5percent. One annuity will pay $2,000 annually, starting today, for 20 years. The second annuity will pay annually, starting one year from today, for 20 years. What is the annual payment for the second annuity?A. $2,225B. $2,075C. $2,000D. $2,130E. $2,40530.Kay owns two annuities that will each pay $500 a month for the next 12 years. One payment isreceived at the beginning of each month while the other is received at the end of each month. Ata discount rate of 7.25 percent, compounded monthly, what is the difference in the present valuesof these annuities?A. $289.98B. $265.42C. $299.01D. $308.00E. $312.5031.What is the future value of $845 a year for seven years at an interest rate of 11.3 percent?A. $6,683.95B. $6,075.69C. $8,343.51D. $8,001.38E. $8,801.9132.What is the future value of $3,100 a year for six years at interest rate of 8.9 percent?A. $20,255.40B. $26,847.26C. $27,134.16D. $23,263.57E. $24,414.6733.Janet saves $3,000 a year at an interest rate of 4.2 percent. What will her savings be worth at theend of 35 years?A. $229,317.82B. $230,702.57C. $230,040.06D. $234,868.92E. $236,063.6634.You plan to save $2,400 a year and earn an average rate of interest of 5.6 percent. How muchmore will your savings be worth at the end of 40 years if you save at the beginning of each year rather than at the end of each year?A. $17,822.73B. $18,821.10C. $18,911.21D. $19,103.04E. $18,115.3135.You borrow $12,600 to buy a car. The terms of the loan call for monthly payments for five yearsat an interest rate of 4.65 percent, compounded monthly. What is the amount of each payment?A. $253.22B. $243.73C. $230.62D. $235.76E. $233.0436.You borrow $199,000 to buy a house. The mortgage rate is 5.5 percent, compounded monthly.The loan period is 30 years, and payments are made monthly. If you pay for the house according to the loan agreement, how much total interest will you pay?A. $218,086B. $198,161C. $207,764D. $211,086E. $185,05937.Luis has a management contract which grants him a lump sum payment of $20 million be paidupon the completion of his first five years of service. The company wants to set aside an equal amount of funds each year to cover this anticipated cash outflow. The company can earn 4.5 percent on these funds. How much must the company set aside each year for this purpose?A. $3,775,042.93B. $3,798,346.17C. $3,801,033.67D. $3,655,832.79E. $4,038,018.2238.On the day you retire, you have $389,900 in your retirement savings. You expect to earn 4.5percent, compounded monthly, and live 24 more years. How much can you withdraw from your savings each month during your retirement if you plan to die on the day you spend your last penny?A. $2,181.96B. $2,092.05C. $2,398.17D. $2, 072.00E. $2,216.2939.Donaldson’s purchased some property for $1.2 million, paid 25 percent down in cash, andfinanced the balance for 12 years at 7.2 percent, compounded monthly. What is the amount of each monthly mortgage payment?A. $8,440.01B. $8,978.26C. $9,351.66D. $9,399.18E. $9,513.6740.Assume you graduate with $31,300 in student loans at an interest rate of 5.25 percent,compounded monthly. If you want to have this debt paid in full within three years, how much must you pay each month?A. $871.30B. $873.65C. $876.79D. $941.61E. $980.4041.You are buying a car for $7,500, paying $900 down in cash, and financing the balance for 24months at 6.5 percent, compounded monthly. What is the amount of each monthly loanpayment?A. $318.64B. $294.01C. $302.02D. $264.78E. $245.0942.You want to purchase an annuity that will pay you $1,200 a quarter for 15 years and earn a returnof 5.5 percent, compounded quarterly. What is the most you should pay to purchase thisannuity?A. $52,988.16B. $48,811.20C. $47,455.33D. $48,450.67E. $52,806.3043.A car dealer is willing to lease you a car for $319 a month for 60 months. Payments are due onthe first day of each month starting with the day you sign the lease contract. If your cost of money is 4.9 percent, compounded monthly, what is the current value of the lease?A. $17,882.75B. $17,906.14C. $17,014.34D. $16,235.42E. $16,689.5444.Sara is the recipient of a trust that will pay her $500 on the first day of each month, startingimmediately and continuing for 40 years. What is the value of this inheritance today if theapplicable discount rate is 7.3 percent, compounded monthly?A. $76,811.30B. $67,557.52C. $89,204.04D. $78,192.28E. $80,006.0945.Beatrice invests $1,000 in an account that pays 5 percent simple interest. How much more couldshe have earned over a 10-year period if the interest had compounded annually?A. $132.45B. $135.97C. $128.89D. $117.09E. $121.6746.Nu-Tools plans to set aside an equal amount of money each year, starting today, so that it willhave $25,000 saved at the end of three years. If the firm can earn 4.7 percent, how much does it have to save annually?A. $7,596.61B. $7,689.16C. $8,004.67D. $8,414.14E. $8,333.3347.Starting today, Alicia is going to contribute $100 a month to her retirement account. Her employermatches her contribution by 50 percent. If these contributions remain constant, and she earns a monthly rate of .55 percent, how much will her savings be worth 40 years from now?A. $399,459.44B. $300,456.74C. $349,981.21D. $299,189.16E. $354,087.8848.An annuity costs $70,000 today, pays $3,500 a year, and earns a return of 4.5 percent. What isthe length of the annuity time period?A.54.96 yearsB.49.48 yearsC.52.31 yearsD.43.08 yearsE.48.00 years49.You are borrowing $5,200 at 7.8 percent, compounded monthly. The monthly loan payment is$141.88. How many loan payments must you make before the loan is paid in full?A. 30B. 36C. 40D. 42E. 4850.You are retired, have $264,500 in your savings, withdraw $2,000 each month, and earn 4.5percent, compounded monthly. How long will it be until you run out of money?A. 13.67 yearsB. 15.25 yearsC. 22.08 yearsD. 13.02 yearsE. 18.78 years51.A project is expected to produce cash flows of $48,000, $39,000, and $15,000 over the next threeyears, respectively. After three years, the project will be worthless. What is the present value of this project if the applicable discount rate is 15.25 percent?A. $89,201.76B. $80,809.09C. $73,457.96D. $97,808.17E. $93,132.4852.You are considering two savings options that each provide a rate of return of 4.65 percent. Thefirst option requires annual savings of $2,000, $2,500, and $3,000 over the next three years, respectively, with the first deposit due one year from today. The other option is to save one lump sum amount today. If you want to have the same balance in your savings at the end of the three years, regardless of the savings method you select, how much do you need to save today if you select the lump sum option?A. $6,811.50B. $6,791.42C. $7,128.23D. $6,607.23E. $7,500.0053.You are considering two insurance settlement offers. The first offer includes annual payments of$36,000, $42,000, and $50,000 over the next three years, respectively, with the first payment being made one year from today. The other offer is the payment of one lump sum amount today.The relevant discount rate is 7 percent. What is the minimum amount you should accept today if you are to select the lump sum offer?A. $119,877.67B. $111,144.18C. $105,000.10D. $118,924.27E. $114,556.8854.You are considering a 3-year job offer. The job offers an annual salary of $48,000, $51,000, and$55,000 a year for the next three years, respectively. The offer also includes a starting bonus of $2,500 payable immediately. What is this offer worth to you today at a discount rate of 6.5percent?A. $129,640.14B. $134,383.56C. $132,283.56D. $138,066.75E. $130,983.5655.You are considering a project with projected annual cash inflows of $32,200, $41,800, $22,900for the next three years, respectively. What is the value of the project today at a discount rate of14 percent?A. $86,487.47B. $75,866.20C. $77,103.18D. $81,292.25E. $66,549.3056.You expect an investment to return $11,300, $14,600, $21,900, and $38,400 annually over thenext four years, respectively. What is this investment worth to you today if you desire a rate of return of 16.5 percent?A. $64,253.91B. $58,700.89C. $63,732.41D. $55,153.57E. $59,928.1657.A 3-year project is expected to produce a cash flow of $82,400 in the first year and $148,600 inthe second year. The project has a present value of $303,764.34 at a discount rate of 12.75 percent. What is the expected cash flow in the third year of the project?A. $163,100B. $163,800C. $164,900D. $164,400E. $163,70058.Two years ago, the Fun Center deposited $3,200 in an investment account for the purpose ofbuying new equipment three years from today. Today, it is adding another $5,000 to this account.It plans on making a final deposit of $3,500 to the account next year. How much will be available when it is ready to buy the equipment, assuming the account earns a rate of return of 6.85percent?A. $13,619.29B. $13,430.84C. $12,746.17D. $14,194.54E. $14,552.2159.Anna has $38,654 in a savings account that pays 2.3 percent interest. Assume she withdraws$10,000 today and another $10,000 one year from today. If she waits and withdraws theremaining entire balance four years from today, what will be the amount of that withdrawal?A. $20,916.78B. $20,109.08C. $20,676.53D. $19,341.02E. $19,608.0760.Theo is depositing $1,300 today in an account with an expected rate of return of 8.1 percent. If hedeposits an additional $3,200 two years from today, and $4,000 three years from today, what will his account balance be ten years from today?A. $14,044.89B. $16,412.31C. $15,182.53D. $15,699.54E. $17,741.7161.Leo received $7,500 today and will receive another $5,000 two years from today. He will investthese funds when he receives them and expects to earn a rate of return of 11.5 percent. What value does he expect his investments to have five years from today?A. $18,758.04B. $18,806.39C. $19,856.13D. $20,314.00E. $19,904.3662.Suzette is receiving $10,000 today, $15,000 one year from today, and $25,000 four years fromtoday. She will immediately invest these funds for retirement. If she earns 9.6 percent on her investments, how much will she have in savings 30 years from today?A. $586,124.93B. $591,414.14C. $646,072.91D. $620,008.77E. $641,547.3963.BJ’s goal is to have $50,000 saved at the end of Year 5. At the end of Year 2, they can add$7,500 to their savings but they want to deposit the remainder they need to reach their goal today, Year 0, as a lump sum deposit. If they can earn 4.5 percent, how much must they deposit today? A. $31,867.74A. A NSB. $33,254.58B. $33,108.09C. $34,276.34D. $34,642.2864.The government imposed a fine on the Not-So-Legal Company. The fine calls for a payment of $100,000 today, $150,000 one year from today, and $200,000 two years from today. Thegovernment will hold the funds until the final payment is collected and then donate the entire amount to charity. The government has agreed to pay annual interest of 3 percent on the held funds. How much will be donated to charity in two years?A. $475,000.00B.$460,590.00C. $447,174.76D. $451,050.05E.$474,407.7065.Benson’s established a trust fund that provides $125,000 in college scholarships each year. Thetrust fund earns a rate of return of 6.15 percent and distributes only its annual income. How much money did Benson’s contribute to establish the trust fund?A.$2,291,613.13B.$2,032,520.33C.$2,150,000.00D.$2,018,970.44E.$1,987,408.1566.A preferred stock pays an annual dividend of $6.50 a share and has an annual rate of return of7.35 percent. What is the stock price?A. $74.50B. $71.78C. $92.09D.$88.44E.$77.7867.You want to establish a trust fund that will provide $50,000 a year forever for your heirs. If thefund can earn a guaranteed rate of return of 4.5 percent, how much must you deposit in a lump sum to establish this trust? This will be the only deposit you make to the fund.A.$1,333,333.33B.$2,250,000.00C.$1,250,000.00D.$1,666,666.67E.$1,111,111.1168.You just paid $525,000 for a security that will pay you and your heirs $25,000 a year forever.What rate of return will you earn?A. 4.95%B. 4.39%C. 4.76%D. 5.00%E. 4.50%69.Anna’s grandmother established a trust and deposited $250,000 into it. The trust pays aguaranteed 4.25 percent rate of return. Anna will receive all of the interest earnings on an annual basis and a charity will receive the principal amount at Anna’s passing. How much incom e will Anna receive each year?A. $10,000B. $8,500C. $12,400D.$10,625E.$12,75070.The preferred stock of ABC Co. offers a rate of return of 7.87 percent. The stock is currentlypriced at $63.53 per share. What is the amount of the annual dividend?A. $5.20B. $5.00C. $4.60D. $5.50E. $6.0071.Your credit card company charges you 1.35 percent per month. What is the annual percentagerate on your account?A. 16.45%B. 16.30%C. 16.39%D. 16.20%E. 16.56%72.What is the annual percentage rate on a loan that charges interest of 1.65 percent per quarter?A. 6.50%B. 6.45%C. 6.54%D. 6.60%E. 6.72%73.A credit card compounds interest monthly and has an effective annual rate of 12.67 percent.What is the annual percentage rate?A. 12.35%B. 12.00%C. 11.99%D. 11.87%E. 11.93%74.What is the effective annual rate if your credit card charges you 10.64 percent compoundeddaily? (Assume a 365-day year.)A. 10.79%B. 11.22%C. 11.95%D. 11.48%E. 12.01%75.Taylor’s Hardware offers credit at an APR of 14.9 percent and compounds interest monthly. Whatis the actual rate of interest they are charging?A. 13.97%B. 14.90%C. 15.48%D. 15.96%E. 16.10%76.The pawn shop adds 2 percent to loan balances for every two weeks a loan is outstanding. Whatis the effective annual rate they are charging?A. 79.97%B. 73.08%C. 51.21%D. 67.34%E. 83.43%77.You have $2,500 to deposit into a savings account. The five banks in your area offer the followingrates. In which bank should you deposit your savings?A. B ank A: 3.75%, compounded annuallyB. B ank B: 3.69%, compounded monthlyC. B ank C: 3.70% compounded semi-annuallyD. B ank D: 3.67% compounded continuouslyE. B ank E; 3.65% compounded quarterly78.What is the effective annual rate of 13.52 percent compounded continuously?A. 14.23%B. 13.84%C. 13.97%D. 14.48%E. 14.56%79.What is the effective annual rate of 10.25% compounded continuously?A. 10.98%B. 11.11%C. 10.79%D. 11.04%E. 10.86%80.The Smart Bank wants to be competitive based on quoted loan rates and thus must offer loans atan annual percentage rate of 7.9 percent. What is the maximum rate the bank can actually earn based on this quoted rate?A. 7.90%B. 8.18%C. 8.20%D. 8.22%E. 8.39%81.Thirty-five years ago, your father invested $2,000. Today that investment is worth $98,407. Whatrate of return has your father earned on his investment?A. 10.94%B. 11.33%C. 10.50%D. 11.77%E. 9.99%82.What is the future value of investing $5,650 for 14 years at a continuously compounded rate of8.6 percent?A. $17,933.54B. $16,685.44C. $19,369.83D. $18,833.85E. $13,183.8583.Assume you could invest $25,000 at a continuously compounded rate of 10 percent. What wouldyour investment be worth at the end of 50 years?A. $2,933,054B. $3,500,824C. $3,911,215D. $3,710,329E. $3,648,02984.A trust has been established to fund scholarships in perpetuity. The next annual distribution willbe $1,200 and future payments will increase by 3 percent per year. What is the value of this trust at a discount rate of 7.4 percent?A. $17,189.19B. $19,960.00C. $27,272.73D. $24,609.11E. $30,388.1885.Stu can purchase a one-bedroom house near his college today for $110,000, including the cost ofsome minor repairs. He expects to be able to resell it in four years for $150,000 if he just puts a little effort into cleaning up the property. At a discount rate of 6.5 percent, what is the expected net present value of this purchase opportunity?A. $3,001.61B. $2,487.43C. $6,598.46D. $7,208.18E. $4,311.02。
公司理财原版英文课件4

$20,000 $9,943.53 (1.15)5
4-14
4.5 Finding the Number of Periods
If we deposit $5,000 today in an account paying 10%, how long does it take to grow to $10,000?
$5.92 > $1.10 + 5×[$1.10×.40] = $3.30
This is due to compounding.
4-12
Future Value and Compounding
$1.10 (1.40) $1.10 (1.40) 4 $1.10 (1.40)3 $1.10 (1.40) 2 $1.10 (1.40)
4-7
Net Present Value
$10,000 NPV $9,500 1.05 NPV $9,500 $9,523.81 NPV $23.81
The present value of the cash inflow is greater than the cost. In other words, the Net Present Value is positive, so the investment should be purchased.
N = number of periods Remember to clear the registers (CLR TVM) after each problem Other calculators are similar in format
4-17
Multiple Cash Flows
公司理财课程大纲

公司理财课程大纲课程名称:公司理财/ Corporate Finance课程编号:241076课程属性:专业教育必修课授课对象:各专业本科生总学时/学分:64/4开课学期:第3学期执笔人:先修课程:财务管理编写日期:一、课程概述公司理财是就公司经营过程中的资金运动进行预测、组织、协调、分析和控制的一种决策与管理活动。
从决策角度来讲,公司理财的决策内容包括投资决策、筹资决策、股利政策和短期财务决策;从管理角度来讲,公司理财的管理职能主要是至对资金筹集和资金投放的管理。
本门课程在财务管理课程内容的基础上,对公司财务决策进行全面、深入的学习。
Corporate Finance is to predict the course of operation on the movement of funds, a decision-making and management activities of the organization, coordination, analysis and control. From the perspective of decision-making, corporate finance including investment decision-making, funding decisions, dividend policy and short-term financial decision-making; from a management point of view, corporate finance management function is mainly to put on fund-raising and fund management. The course is based on the financial management of course content on the company's financial decision-making comprehensive, in-depth study.二、课程目标1.掌握公司理财的基本目标、投资决策、融资决策和利润分配的基本原理和方法;2.熟悉投资评价的各种方法(净现值法、内部收益率法、回收期法、折现回收期法等等)、资本结构的相关理论(权衡理论、信号理论、融资优序理论等);3.学会股票和债券定价、投资收益的估计、长期计划与财务预测;4. 了解公司理财的理论与实践的最新进展,培养学生运用所学理论知识发现、解释现实世界公司财务问题的能力。
{财务管理公司理财}公司理财经典讲义英文版

of the Firm
The Capital Structure Decision
Current
Current Assets
Liabilities
How can the firm
raise the money Fixed Assets for the required
Long-Term Debt
1 Tangible investments?
investments?
McGraw-Hill/Irwin
© 2005 The McGraw-Hill Companies, Inc. All Rights Reserved.
1-4
The Balance-Sheet Model
of the Firm
Total Value of Assets:
Total Firm Value to Investors:
Current
Current Assets
Liabilities Long-Term
Debt
Fixed Assets
1 Tangible
2 Intangible
McGraw-Hill/Irwin
Shareholders’ Equity
© 2005 The McGraw-Hill Companies, Inc. All Rights Reserved.
2750%50%30% DebtDeEbtquity
5705%
Equity
McGraw-Hill/Irwin
If how you slice the pie affects the size of the pie, then the capital structure decision matters.
《公司理财》斯蒂芬A.罗斯..,机械工业出版社 英文课件

19-1
Executive Summary
This chapter looks at how corporations issue securities to the investing public. As the basic procedure for selling debt and equity securities are essentially the same. This chapter focuses on equity.
McGraw-Hill/Irwin
Copyright 2004 by The McGraw-Hill Companies, Inc. All rights reserved.
19-3
19.1 The Public Issue
The Basic Procedure
– Management gets the approval of the Board of Directors. – The firm prepares and files a registration statement with the SEC. – The SEC studies the registration statement during the waiting period. – The firm prepares and files an amended registration statement with the SEC. – If everything is copasetic with the SEC, a price is set and a full-fledged selling effort gets underway.
19-8
Firm Commitment
公司理财(罗斯)第2章(英文)

2-2
Sources of Information
Annual reports Wall Street Journal Internet
2.1 The Balance Sheet 2.2 The Income Statement 2.3 Net Working Capital 2.4 Financial Cash Flow 2.5 The Statement of Cash Flows 2.6 Financial Statement Analysis 2.7 Summary and Conclusions
McGraw-Hill/Irwin Corporate Finance, 7/e 2005 The McGraw-Hill Companies, Inc. All Rights Reserved.
2-6
Debt versus Equity
Generally, when a firm borrows it gives the bondholders first claim on the firm’s cash flow. Thus shareholder’s equity is the residual difference between assets and liabilities.
Total assets
McGraw-Hill/Irwin Corporate Finance, 7/e
$1,879
$1,742
2005 The McGraw-Hill Companies, Inc. All Rights Reserved.
公司理财实务英文版

2 Intangible
Current Liabilities Long-Term
Debt
Shareholders’ Equity
What is 城市轨道交通 urban rail transport
精品ppt模板
The Net Working Capital Investment Decision
• If the value of the firm is less than the amount promised to the debtholders, the shareholders get nothing.
What is 城市轨道交通 urban rail transport
精品ppt模板
Max[0,$X – $F] = $X – $F and the debt holder’s claim is:
Debt holders are promised $F.
Min[$F,$X] = $F. The sum of these is = $X
What is 城市轨道交通 urban rail transport
pie, then the capital structure decision
matters.
What is 城市轨道交通 urban rail transport
精品ppt模板
Firm
Firm issues securities (A)
Invests in assets
(B)
Retained cash flows (F)
• Primary Market
– When a corporation issues securities, cash flows from investors to the firm.
公司理财第四章

• Degree of financial leverage – determined by capital structure decisions
• Cash paid to shareholders – determined by dividend policy decisions
• Understand how capital structure policy and dividend policy affect a firm’s ability to grow
4-2
Chapter Outline
• What Is Financial Planning? • Financial Planning Models: A First
by reasonable amounts – Determine, at a minimum, worst case, normal case, and
best case scenarios
4-5
Role of Financial Planning
• Examine interactions – help management see the interactions between decisions
4-6ห้องสมุดไป่ตู้
Financial Planning Model Ingredients
• Sales Forecast – many cash flows depend directly on the level of sales (often estimated using sales growth rate)
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
4-4
Financial Planning Process
• Planning Horizon - divide decisions into short-run decisions (usually next 12 months) and long-run decisions (usually 2 – 5 years) • Aggregation - combine capital budgeting decisions into one large project • Assumptions and Scenarios
– Dividends are the plug variable, so equity Assets increases at 15% – Dividends = 460 (NI) – 370 (increase in equity)Total = 90 dividends paid
• Case II
4-7
Example: Historical Financial Statements
Gourmet Coffee Inc. Balance Sheet December 31, 2009
Gourmet Coffee Inc.
Income Statement For Year Ended December 31, 2009
Percentage of Sales Approach
• Some items vary directly with sales, while others do not • Income Statement
– Costs may vary directly with sales - if this is the case, then the profit margin is constant – Depreciation and interest expense may not vary directly with sales – if this is the case, then the profit margin is not constant – Dividends are a management decision and generally do not vary directly with sales – this influences additions to retained earnings
4-5
Role of Financial Planning
• Examine interactions – help management see the interactions between decisions • Explore options – give management a systematic framework for exploring its opportunities • Avoid surprises – help management identify possible outcomes and plan accordingly • Ensure feasibility and internal consistency – help management determine if goals can be accomplished and if the various stated (and unstated) goals of the firm are consistent with one another
4-11
Example: Income Statement
Tasha’s Toy Emporium Income Statement, 2009 % of Sales Sales 5,000 Tasha’s Toy Emporium Pro Forma Income Statement, 2010 Sales 5,500
• Balance Sheet
– Initially assume all assets, including fixed, vary directly with sales – Accounts payable will also normally vary directly with sales – Notes payable, long-term debt and equity generally do not vary directly with sales because they depend on management decisions about capital structure – The change in the retained earnings portion of equity will come from the dividend decision
– Make realistic assumptions about important variables – Run several scenarios where you vary the assumptions by reasonable amounts – Determine, at a minimum, worst case, normal case, and best case scenarios
Less: costs
EBT
(3,300)
2,200
Less: costs
EBT Less: taxes (40% of EBT) Net Income Dividends Add. To RE
(3,000)
2,000 (800)
4-6
Financial Planning Model Ingredients
• Sales Forecast – many cash flows depend directly on the level of sales (often estimated using sales growth rate) • Pro Forma Statements – setting up the plan using projected financial statements allows for consistency and ease of interpretation • Asset Requirements – the additional assets that will be required to meet sales projections • Financial Requirements – the amount of financing needed to pay for the required assets • Plug Variable – determined by management deciding what type of financing will be used to make the balance sheet balance • Economic Assumptions – explicit assumptions about the coming economic environment
4-9
Example: Pro Forma Balance Sheet
• Case I
Gourmet Coffee Inc. Pro Forma Balance Sheet Case 1 1,150 Debt Equity 1,150 Total Gourmet Coffee Inc. 460 690 1,150
Gourmet Coffee Inc.
– Revenues will grow Pro Forma Income Statement at 15% For Year Ended 2010 (2,000*1.15) – All items are tied Revenues 2,300 directly to sales, and the current relationships are Less: costs (1,840) optimal – Consequently, all Net Income 460 other items will also grow at 15%
Assets
1000 Debt
Equity
400
Revenues 600 Less: costs 1000 Net Income 2000 (1600) 400
Total
1000 Total
4-8
Example: Pro Forma Income Statement
• Initial Assumptions
• Understand the financial planning process and how decisions are interrelated • Be able to develop a financial plan using the percentage of sales approach • Be able to compute external financing needed and identify the determinants of a firm’s growth • Understand the four major decision areas involved in long-term financial planning • Understand how capital structure policy and dividend policy affect a firm’s ability to grow
4-3
Elements of Financial Planning
• Investment in new assets – determined by capital budgeting decisions • Degree of financial leverage – determined by capital structure decisions • Cash paid to shareholders – determined by dividend policy decisions • Liquidity requirements – determined by net working capital decisions
