2004年江苏专转本高等数学真题(附答案)

合集下载

2004年高考.江苏卷.数学试题及答案

C1
(Ⅲ)求点 P 到平面 ABD1 的距离.
·O
A1
B1
·H
P
D 第 2页（2共 6页）
A
C B
19.制定投资计划时，不仅要考虑可能获得的盈利，而且要考虑可能出现的亏损. 某投资人打算投资甲、乙两个项目. 根据预测，甲、乙项目可能的最大盈利率分别为 100
﹪和 50﹪，可能的最大亏损分别为 30﹪和 10﹪. 投资人计划投资金额不超过 10 万元，要求确保可能的资金亏损不超过 1.8 万元. 问投资人对甲、乙两个项目各投资多少万元，才能使可能的盈利最大？
设实数 a0，a，b 满足 f (a0 ) 0 和 b a λf (a) (Ⅰ)证明 λ 1 ，并且不存在 b0 a0 ，使得 f (b0 ) 0 ； (Ⅱ)证明 (b a0 ) 2 (1 λ2 )(a a0 ) 2 ； (Ⅲ)证明 [ f (b)]2 (1 λ2 )[ f (a)]2 .
先后抛掷 3 次，至少出现一次 6 点向上和概率是
(
)
(A) 5 216
(B) 25 216
(C) 31 216
(D) 91 216
10.函数 f (x) x 3 3x 1 在闭区间[-3，0]上的最大值、最小值分别是
(
)
(A)1，-1
(B)1，-17
(C)3，-17
(D)9，-19
11.设 k>1，f(x)=k(x-1)(x∈R) . 在平面直角坐标系 xOy 中，函数 y=f(x)的图象与 x 轴交于 A
M=N 成立的实数对(a，b)有
(
)
(A)0 个
(B)1 个
(C)2 个
(D)无数多个
二、填空题(4 分×4=16 分)

成人专升本高等数学一真题2004年_真题(含答案与解析)-交互

成人专升本高等数学一真题2004年(总分150, 做题时间90分钟)一、选择题1.SSS_SIMPLE_SINA B C D该题您未回答:х该问题分值: 4答案:D2.SSS_SIMPLE_SINA B C D该题您未回答:х该问题分值: 4答案:B3.SSS_SIMPLE_SINA B C D该题您未回答:х该问题分值: 4答案:B4.SSS_SIMPLE_SINA B C D该题您未回答:х该问题分值: 4答案:C5.SSS_SIMPLE_SINA B C D该题您未回答:х该问题分值: 4答案:A二、填空题6.SSS_FILL该题您未回答:х该问题分值: 4答案:e7.SSS_FILL该题您未回答:х该问题分值: 4答案:8.SSS_FILL该题您未回答:х该问题分值: 4答案:19.SSS_FILL该题您未回答:х该问题分值: 4答案:10.SSS_FILL该题您未回答:х该问题分值: 4答案:11.SSS_FILL该题您未回答:х该问题分值: 4答案:12.SSS_FILL该题您未回答:х该问题分值: 4答案:213.SSS_FILL该题您未回答:х该问题分值: 4答案:14.SSS_FILL该题您未回答:х该问题分值: 4答案:15.SSS_FILL该题您未回答:х该问题分值: 4答案:三、解答题16.SSS_TEXT_QUSTI该题您未回答:х该问题分值: 617.SSS_TEXT_QUSTI该题您未回答:х该问题分值: 618.SSS_TEXT_QUSTI该题您未回答:х该问题分值: 619.SSS_TEXT_QUSTI该题您未回答:х该问题分值: 620.SSS_TEXT_QUSTI该题您未回答:х该问题分值: 621.SSS_TEXT_QUSTI该题您未回答:х该问题分值: 622.SSS_TEXT_QUSTI该题您未回答:х该问题分值: 623.SSS_TEXT_QUSTI该题您未回答:х该问题分值: 624.SSS_TEXT_QUSTI该题您未回答:х该问题分值: 625.SSS_TEXT_QUSTI该题您未回答:х该问题分值: 626.SSS_TEXT_QUSTI该题您未回答:х该问题分值: 1027.SSS_TEXT_QUSTI该题您未回答:х该问题分值: 1028.SSS_TEXT_QUSTI该题您未回答:х该问题分值: 101。

2004年高考.江苏卷.数学试题及答案

时间(小时) 2004年普通高等学校招生全国统一考试数学（江苏卷）一、选择题(5分×12=60分)1.设集合P={1，2，3，4}，Q={R x x x ∈≤,2}，则P ∩Q 等于( )(A){1，2} (B) {3，4} (C) {1} (D) {-2，-1，0，1，2}2.函数y=2cos 2x+1(x ∈R )的最小正周期为( ) (A)2π (B)π (C)π2 (D)π4 3.从4名男生和3名女生中选出4人参加某个座谈会，若这4人中必须既有男生又有女生，则不同的选法共有 ( )(A)140种 (B)120种 (C)35种 (D)34种4.一平面截一球得到直径是6cm 的圆面，球心到这个平面的距离是4cm ，则该球的体积是( ) (A)33π100cm (B) 33π208cm (C) 33π500cm (D) 33π3416cm 5.若双曲线18222=-by x 的一条准线与抛物线x y 82=的准线重合，则双曲线离心率为 ( ) (A)2 (B)22 (C) 4 (D)246.某校为了了解学生的课外阅读情况，随机调查了50名学生，得到他们在某一天各自课外阅读所用时间的数据，结果用右侧的条形图表示. 根据条形图可得这50名学生这一天平均每人的课外阅读时间为 ( )(A)0.6小时 (B)0.9小时 (C)1.0小时 (D)1.5小时7.4)2(x x +的展开式中x 3的系数是 ( )(A)6 (B)12 (C)24 (D)488.若函数)1,0)((log ≠>+=a a b x y a 的图象过两点(-1，0)和(0，1)，则 ( )(A)a=2,b=2 (B)a= 2 ,b=2 (C)a=2,b=1 (D)a= 2 ,b= 29.将一颗质地均匀的骰子（它是一种各面上分别标有点数1，2，3，4，5，6的正方体玩具）先后抛掷3次，至少出现一次6点向上和概率是 ( )(A)5216 (B)25216 (C)31216 (D)9121610.函数13)(3+-=x x x f 在闭区间[-3，0]上的最大值、最小值分别是 ( )(A)1，-1 (B)1，-17 (C)3，-17 (D)9，-1911.设k>1，f(x)=k(x-1)(x ∈R ) . 在平面直角坐标系xOy 中，函数y=f(x)的图象与x 轴交于A点，它的反函数y=f -1(x)的图象与y 轴交于B 点，并且这两个函数的图象交于P 点. 已知四边形OAPB 的面积是3，则k 等于 ( )(A)3 (B)32 (C)43 (D)6512.设函数)(1)(R x xx x f ∈+-=，区间M=[a ，b](a<b)，集合N={M x x f y y ∈=),(}，则使M=N 成立的实数对(a ，b)有 ( )(A)0个 (B)1个 (C)2个 (D)无数多个二、填空题(4分×4=16分)13.二次函数y=ax 2+bx+c(x ∈R )的部分对应值如下表：则不等式ax +bx+c>0的解集是_______________________.14.以点(1，2)为圆心，与直线4x+3y-35=0相切的圆的方程是________________.15.设数列{a n }的前n 项和为S n ，S n =2)13(1-n a (对于所有n ≥1)，且a 4=54，则a 1的数值是_______________________.16.平面向量,中，已知a =(4，-3)=1，且b a ⋅=5，则向量b =__________.三、解答题(12分×5+14分=74分)17.已知0<α<2π，tan 2α+cot 2α=25，求sin(3πα-)的值. 18.在棱长为4的正方体ABCD-A 1B 1C 1D 1中，O 是正方形A 1B 1C 1D 1的中心，点P 在棱CC 1上，且CC 1=4CP.(Ⅰ)求直线AP 与平面BCC 1B 1所成的角的大小（结果用反三角函数值表示）；(Ⅱ)设O 点在平面D 1AP 上的射影是H ，求证：D 1H ⊥AP ； (Ⅲ)求点P 到平面ABD 1的距离.· B 1 P D A 1 C 1 D 1O H ·19.制定投资计划时，不仅要考虑可能获得的盈利，而且要考虑可能出现的亏损.某投资人打算投资甲、乙两个项目. 根据预测，甲、乙项目可能的最大盈利率分别为100﹪和50﹪，可能的最大亏损分别为30﹪和10﹪. 投资人计划投资金额不超过10万元，要求确保可能的资金亏损不超过1.8万元. 问投资人对甲、乙两个项目各投资多少万元，才能使可能的盈利最大？20.设无穷等差数列{a n }的前n 项和为S n .(Ⅰ)若首项=1a 32 ，公差1=d ，求满足2)(2k kS S =的正整数k ； (Ⅱ)求所有的无穷等差数列{a n }，使得对于一切正整数k 都有2)(2k k S S=成立.21.已知椭圆的中心在原点，离心率为12，一个焦点是F （-m,0）(m 是大于0的常数). (Ⅰ)求椭圆的方程；(Ⅱ)设Q 是椭圆上的一点，且过点F 、Q 的直线l 与y 轴交于点M. =，求直线l 的斜率.22.已知函数))((R x x f ∈满足下列条件：对任意的实数x 1，x 2都有)]()()[()(λ2121221x f x f x x x x --≤-和2121)()(x x x f x f -≤-，其中λ是大于0的常数.设实数a 0，a ，b 满足 0)(0=a f 和)(λa f a b -=(Ⅰ)证明1λ≤，并且不存在00a b ≠，使得0)(0=b f ；(Ⅱ)证明20220))(λ1()(a a a b --≤-；(Ⅲ)证明222)]()[λ1()]([a f b f -≤.2004年普通高等学校招生全国统一考试数学（江苏卷）参考答案一、选择题ABDCA BCADC BA二、填空题13、{2x x <-或3}x >14、22(1)(1)25x y -+-=15、216、43(,)55b =-三、解答题17、解：由题意可知4sin 5α=，sin()3πα∴-=18、解（1）arctan APB ∠=（2）略（319、解：10318x y x y +≤⎧⎨+≤⎩，设0.5z x y =+当46x y =⎧⎨=⎩时，z 取最大值7万元20、解：（1）4k =（2）100a d =⎧⎨=⎩或112a d =⎧⎨=⎩或110ad =⎧⎨=⎩21、解：（1）2222143x y m m +=（2）k =±或022、解：（1）不妨设12x x >，由[]2121212()()()()x x x x f x f x λ-≤-⋅-可知12()()0f x f x ->，()f x ∴是R 上的增函数∴不存在00b a ≠，使得0()0f b =又[]2212121212()()()()()x x x x f x f x x x λ-≤-⋅-≤-1λ∴≤（2）要证：222000()(1)()b a a a λ-≤--即证：2200()()2()()a a f a f a a a λ⎡⎤-+≤-⎣⎦(*) 不妨设0a a >，由[]2121212()()()()x x x x f x f x λ-≤-⋅-得00()()()f a f a a a λ-≥-，即0()()f a a a λ≥-，则2002()()2()f a a a a a λ-≥- （1）由1212()()f x f x x x -≤-得00()()f a f a a a -≤- 即0()f a a a ≤-，则22200()()2()a a f a a a λλ⎡⎤-+≤-⎣⎦ （2）由（1）（2）可得2200()()2()()a a f a f a a a λ⎡⎤-+≤-⎣⎦222000()(1)()b a a a λ∴-≤--（3）220[()]()f a a a ≤-,22220(1)[()](1)()f a a a λλ∴-≤--220[()]()f b b a ≤-又由（2）中结论222000()(1)()b a a a λ-≤--222[()](1)[()]f b f a λ∴≤-。

江苏专转本2001-2011年数学历年真题

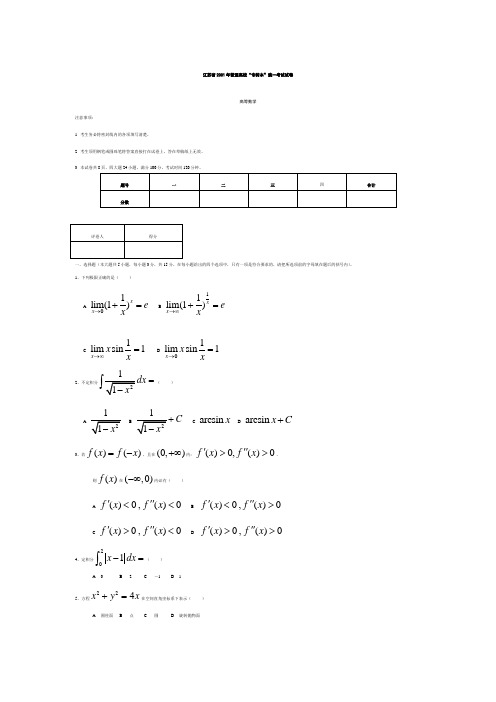
江苏省2001年普通高校“专转本”统一考试试卷高等数学注意事项：1. 考生务必将密封线内的各项填写清楚。

2. 考生须用钢笔或圆珠笔将答案直接打在试卷上，答在草稿纸上无效。

3. 本试卷共8页，四大题24小题，满分100分，考试时间120分钟。

题号一二三四合计分数评卷人得分一、选择题（本大题共5小题，每小题3分，共15分，在每小题给出的四个选项中，只有一项是符合要求的，请把所选项前的字母填在题后的括号内）。

1、下列极限正确的是（）A. 01lim(1)x x e x→+= B. 11lim(1)x x e x →∞+=C.1lim sin1x x x →∞= D. 01lim sin 1x x x→=2、不定积分211dx x=-⎰（）A.211x- B.211C x+- C. arcsin x D. arcsin x C +3、若()()f x f x =-，且在(0,)+∞内：()0,()0f x f x '''>>，则()f x 在(,0)-∞内必有（）A.()0,()0f x f x '''<< B. ()0,()0f x f x '''<> C.()0,()0f x f x '''>< D. ()0,()0f x f x '''>>4、定积分21x dx -=⎰（）A. 0B. 2C. －1D. 15、方程224x y x +=在空间直角坐标系下表示（）A. 圆柱面B. 点C. 圆D. 旋转抛物面评卷人得分二、填空题（本大题共5小题，每小题3分，共15分，请把正确答案的结果填在划线上）。

6、设参数方程为22tx tey t t⎧=⎪⎨=+⎪⎩；则0t dy dx == 。

7、微分方程6130y y y '''-+=的通解为：。

2004年江苏省普通高校“专转本”统一考试高等数学参考答案

2004年江苏省普通高校“专转本”统一考试高等数学参考答案1—6 A BC B A D 7、1-e 8、32241-+==-z y x 9、!n 10、C x +4arcsin 4111、dx y x f dy dx y x f dy yy⎰⎰⎰⎰-+2021010),(),( 12、()3,1-13、间断点为πk x =，Z k ∈，当0=x 时，1sin lim)(lim 00==→→xxx f x x ，为可去间断点；当πk x =，0≠k ，Z k ∈时，∞=→xxx sin lim0，为第二类间断点.14、原式=2411221lim 12)sin 1(tan lim 12sin tan lim 3)sin (tan lim320303040=⋅=-=-=-→→→→⎰xx x x x x x x x x dt t t x x x xx 15、0=x 代入原方程得1)0(=y ，对原方程求导得0''=--y xe e y y y ，对上式求导并将0=x 、1=y 代入，解得：22''e y =.16、因为)(x f 的一个原函数为x e x，所以2')1()(x e x x e x f xx -=⎪⎪⎭⎫ ⎝⎛=， ⎰dx x xf )2('⎰⎰==)2(21)2()2(21'x xdf x d x xf ⎰-=dx x f x xf )2(21)2(21 Cx e x e x x x d x f x xf x x +--=-=⎰88)12()2()2(41)2(21222C e x x x+-=241 17、2arctan 2112)1(2111112122π==+=+-=-∞++∞+∞+∞⎰⎰⎰t dt t dt t t t x t dx x x18、y f f xz⋅+=∂∂'2'1； []x f f y f x f f yx z ⋅+-⋅++⋅+-⋅=∂∂∂''22''21'2''12''112)1()1( ''22''21''12''11'2xyf yf xf f f +-+-=19、原式dy y y dx y y dy dxdy y yy y D⎰⎰⎰⎰⎰-===1010sin )1(sin sin 2 1sin 1cos cos )1(110-=--=⎰ydy y y20、n nn n x x x x f 4)2()1(41421141241)(0--=-+⋅=-+=∑∞=，)42(<-x 21、证明：令x t -=π，⎰⎰⎰-=---=ππππππ0)(sin )()(sin()()(sin dt t f t dt t f t dx x xf⎰⎰-=πππ0)(sin )(sin dx x xf dx x f故⎰⎰=πππ)(sin 2)(sin dx x f dx x xf ，证毕.4)arctan(cos 2cos 1sin 2cos 1sin 200202ππππππ=-=+=+⎰⎰x dx x x dx xx x 22、等式两边求导的)(2)('x f x x xf +=即x x xf x f 2)()('=-且1)0(-=f ，x p -=，x q 2=，⎰-=22xpdx ，22e pdxee -=⎰，22x pdxe e =⎰-，222222x x pdxedx xqdx qe ---==⎰⎰⎰所以2222222)2()(x x x Ce eC ex f +-=+-=--，由1)0(-=f ，解得1=C ，222)(x ex f +-=23、设污水厂建在河岸离甲城x 公里处，则22)50(40700500)(x x x M -++=，500≤≤x ，0)50(40)50(22170050022'=-+-⨯⨯+=x x M解得650050-=x （公里），唯一驻点，即为所求.。

01—10年江苏专转本数学真题(附答案)

2001年江苏省普通高校“专转本”统一考试高等数学一、选择题（本大题共5小题，每小题3分，共15分）1、下列各极限正确的是（）A 、e xxx =+→)11(lim 0B 、e xx x =+∞→1)11(limC 、11sinlim =∞→x x x D 、11sin lim 0=→xx x2、不定积分=-⎰dx x211 （）A 、211x-B 、c x+-211C 、x arcsinD 、c x +arcsin3、若)()(x f x f -=，且在[)+∞,0内0)('>x f 、0)(''>x f ，则在)0,(-∞内必有（）A 、0)('<x f ,0)(''<x f B 、0)('<x f ,0)(''>x f C 、0)('>x f ,0)(''<x f D 、0)('>x f ,0)(''>x f4、=-⎰dx x 21 （）A 、0B 、2C 、－1D 、15、方程x y x 422=+在空间直角坐标系中表示（） A 、圆柱面B 、点C 、圆D 、旋转抛物面二、填空题（本大题共5小题，每小题3分，共15分）6、设⎩⎨⎧+==22tt y te x t ，则==0t dx dy7、0136'''=+-y y y 的通解为 8、交换积分次序=⎰⎰dy y x f dx x x220),(9、函数yx z =的全微分=dz10、设)(x f 为连续函数，则=+-+⎰-dx x x x f x f 311])()([三、计算题（本大题共10小题，每小题4分，共40分） 11、已知5cos)21ln(arctan π+++=xx y ，求dy .12、计算xx dte x xt x sin lim202⎰-→.等价无穷小，洛必达13、求)1(sin )1()(2--=x x xx x f 的间断点，并说明其类型.x 分别为0，1，-1时化简求极限14、已知x y x y ln 2+=，求1,1==y x dxdy.15、计算dx ee xx⎰+12. 16、已知⎰∞-=+02211dx x k ，求k 的值. 17、求x x y y sec tan '=-满足00==x y 的特解.18、计算⎰⎰Ddxdy y2sin ，D 是1=x 、2=y 、1-=x y 围成的区域.19、已知)(x f y =过坐标原点，并且在原点处的切线平行于直线032=-+y x ，若b ax x f +=2'3)(，且)(x f 在1=x 处取得极值，试确定a 、b 的值，并求出)(x f y =的表达式.20、设),(2y x x f z =，其中f 具有二阶连续偏导数，求x z∂∂、yx z ∂∂∂2.四、综合题（本大题共4小题，第21小题10分，第22小题8分，第23、24小题各6分，共30分） 21、过)0,1(P 作抛物线2-=x y 的切线，求（1）切线方程；（2）由2-=x y ，切线及x 轴围成的平面图形面积；（3）该平面图形分别绕x 轴、y 轴旋转一周的体积。

江苏省专转本高等数学试题题型分类整理

江苏省普通高校“专转本”统一考试高等数学专转本高数试卷结构知识分类与历年真题●函数、极限和连续●一元函数微分学●一元函数积分学●向量代数与空间解析几何●多元函数微积分●无穷级数●常微分方程时间排序与参考答案◆2004年高等数学真题参考答案◆2005年高等数学真题参考答案◆2006年高等数学真题参考答案◆2007年高等数学真题参考答案◆2008年高等数学真题参考答案◆2009年高等数学真题参考答案◆2010年高等数学真题参考答案◆2011年高等数学真题参考答案◆2012年高等数学真题参考答案◆2013年高等数学真题参考答案江苏省普通高校“专转本”统一考试高等数学试卷结构全卷满分150分一、单选题（本大题共6小题，每小题4分，满分24分）二、填空题（本大题共6小题，每小题4分，满分24分）三、解答题（本大题共8小题，每小题8分，满分64分）四、综合题（本大题共2小题，每小题10分，满分20分）五、证明题（本大题共2小题，每小题9分，满分18分）知识分类与历年真题一、函数、极限和连续（一）函数（0401）[](]333,0()0,2x x f x x x ⎧∈-⎪=⎨-∈⎪⎩是（） A.有界函数 B.奇函数 C.偶函数 D.周期函数（0801）设函数)(x f 在),(+∞-∞上有定义，下列函数中必为奇函数的是（）A.()y f x =-B.)(43x f x y = C.()y f x =-- D.)()(x f x f y -+= （二）极限（0402）当0→x 时，x x sin 2-是关于x 的（）A.高阶无穷小B.同阶无穷小C.低阶无穷小D.等价无穷小（0407）设xx x x f ⎪⎭⎫⎝⎛++=32)(，则=∞→)(lim x f x ．（0601）若012lim 2x x f x →⎛⎫ ⎪⎝⎭=，则0lim 3x xx f →=⎛⎫ ⎪⎝⎭（） A.21 B.2C.3D.31 （0607）已知0→x 时，(1cos )a x ⋅-与x x sin 是等价无穷小，则=a ．（0613)计算x →．（0701）若0(2)lim2x f x x→=，则1lim 2x xf x →∞⎛⎫= ⎪⎝⎭（） A.41B.21 C.2D.4（0702）已知当0→x 时，)1ln(22x x +是x n sin 的高阶无穷小，而x nsin 又是x cos 1-的高阶无穷小，则正整数=n （） A.1B.2C.3D.4（0813）求极限：32lim xx x x →∞-⎛⎫⎪⎝⎭．（0901）已知22lim32x x ax bx →++=-，则常数b a ,的取值分别为（） A.2,1-=-=b a B.0,2=-=b aC.0,1=-=b aD.1,2-=-=b a（0907）已知lim 2xx x x C →∞⎛⎫= ⎪-⎝⎭，则常数=C ．（1001）设当0x →时，()sin f x x x =-与()ng x ax =是等价无穷小，则常数,a n 的值为 ( ) A.1,36a n == B.1,33a n == C.1,412a n == D.1,46a n == （1007） 1lim 1xx x x →∞+⎛⎫= ⎪-⎝⎭．（1101）当0→x 时，函数1)(--=x e x f x是函数2)(x x g =的( )A.高阶无穷小B.低阶无穷小C.同阶无穷小D.等价无穷小（1107）已知22lim kxx x e x →∞-⎛⎫= ⎪⎝⎭，则=k _________．（1201）极限1sin 3lim 2sinx x x x x →∞⎛⎫+= ⎪⎝⎭（） A.0 B.2 C.3D.5（1301）当0x →时，函数()ln(1)f x x x =+-是函数2()g x x =的（） A.高阶无穷小 B.低阶无穷小C.同阶无穷小D.等价无穷小（1310）设10lim xx a x e a x →+⎛⎫=⎪-⎝⎭，则常数a = ．（三）连续（0413）求函数xxx f sin )(=的间断点，并判断其类型．（0501）0=x 是xx x f 1sin )(=的（） A.可去间断点B.跳跃间断点C.第二类间断点D.连续点（0513）设()2sin 0()0f x xx F x xa x +⎧≠⎪=⎨⎪=⎩在R 内连续，并满足0)0(=f ，(0)6f '=，求a ．（0602）函数21sin 0()00x x f x xx ⎧≠⎪=⎨⎪=⎩在0x =处（） A.连续但不可导B.连续且可导C.不连续也不可导D.可导但不连续（0608）若A x f x x =→)(lim 0，且)(x f 在0x x =处有定义，则当=A 时，)(x f 在0x x =处连续．（0707）设函数1(1)0()20x kx x f x x ⎧⎪+≠=⎨⎪=⎩，在点0=x 处连续，则常数=k ．（0807）设函数21()(1)x f x x x -=-，则其第一类间断点为．（0808）设函数0()tan 30a x x f x x x x+≥⎧⎪=⎨<⎪⎩在点0=x 处连续，则a ＝．（0902）已知函数423)(22-+-=x x x x f ，则2=x 为)(x f 的（）A.跳跃间断点B.可去间断点C.无穷间断点D.震荡间断点（1123）设210arctan ()1010sin 2ax axe x ax x x xf x x e x x ⎧---<⎪⎪⎪==⎨⎪-⎪>⎪⎩，问常数为何值时：（1）0=x 是函数)(x f 的连续点？（2）0=x 是函数)(x f 的可去间断点？（3）0=x 是函数)(x f 的跳跃间断点？（1202）设()2(2)sin ()4x xf x x x -⋅=⋅-，则函数)(x f 的第一类间断点的个数为（） A.0 B.1C.2D.3（1207）要使函数()1()12xf x x =-在点0=x 处连续，则需补充定义(0)f =_________．（1303）设sin 20()0xx x f x x ⎧<⎪⎪=⎨>，这点0x =是函数()f x 的（）A.跳跃间断点B.可去间断点C.无穷间断点D.连续点（1307）设1sin0()0x x f x xa x ⎧≠⎪=⎨⎪=⎩在点0x =处连续，则常数a = ．二、一元函数微分学（一）导数与微分（0403）直线L 与x 轴平行且与曲线xe x y -=相切，则切点的坐标是（） A.()1,1B.()1,1-C.()0,1-D.()0,1（0409）设()(1)(2)()f x x x x x n =+++，N n ∈，则=)0('f ．（0415）设函数)(x y y =由方程1=-yxe y 所确定，求22d d x yx=的值．（0502）若2=x 是函数1ln 2y x ax ⎛⎫=-+ ⎪⎝⎭的可导极值点，则常数=a （） A.1-B.21C.21- D.1 （0514）设函数)(x y y =由方程cos sin cos x t y t t t =⎧⎨=-⎩所确定，求d d y x 、22d d yx ．（0614）若函数)(x y y =是由参数方程2ln (1)arctan x t y t t⎧=+⎨=-⎩所确定，求d d y x 、22d d yx ．（0708）若直线m x y +=5是曲线232++=x x y 的一条切线，则常数=m ．（0714）设函数)(x y y =由方程xy e e yx=-确定，求d d x yx=、22d d x y x =．（0802）设函数)(x f 可导，则下列式子中正确的是（） A.0(0)()lim(0)x f f x f x →-'=- B.000(2)()lim ()x f x x f x f x x→+-'=C.0000()()lim ()x f x x f x x f x x ∆→+∆--∆'=∆D.0000()()lim 2()x f x x f x x f x x∆→-∆-+∆'=∆ （0814）设函数)(x y y =由参数方程sin 1cos x t t y t =-⎧⎨=-⎩（2t n π≠，n Z ∈）所决定，求d d y x 、22d d y x ．（0903）设函数00()1sin 0x f x x x x α≤⎧⎪=⎨>⎪⎩在点0=x 处可导，则常数α的取值范围为（） A.10<<αB.10≤<αC.1>αD.1≥α（0914）设函数)(x y y =由参数方程2ln (1)23x t y t t =+⎧⎨=+-⎩所确定，d d y x 、22d d yx ．（0923）已知函数0()10x e x f x x x -⎧<=⎨+≥⎩，证明函数)(x f 在点0=x 处连续但不可导．（1008）.若(0)1f '=，则0()()limx f x f x x→--= ．（1014）设函数()y y x =由方程2x yy ex ++=所确定，求d d y x 、22d d yx ．（1022）设()0()1x x f x xx ϕ⎧≠⎪=⎨⎪=⎩，其中函数()x ϕ在0x =处具有二阶连续导数，且(0)0ϕ=，(0)1ϕ'=，证明：函数()f x 在0x =处连续且可导．（1102）设函数)(x f 在点0x 处可导，且4)()(lim 000=+--→hh x f h x f h ，则=')(0x f ( )A.4-B.2-C.2D.4（1110）设函数x y arctan=，则1d x y==_____________．（1114）设函数)(x y y =由参数方程⎪⎩⎪⎨⎧=++=22ty e tt x y 所确定，求d d y x ．（1208）设函数()22221x y x x x e =⋅+++，则=)0()7(y________．（1209）设xy x =（0x >），则函数y 的微分=dy ___________．（1214）设函数)(x y y =由参数方程⎪⎩⎪⎨⎧+=-=tt y tt x ln 212所确定，求d d y x 、22d d y x ．（1304）设1y f x ⎛⎫= ⎪⎝⎭，其中f 具有二阶导数，则22d d y x =（）A.231121f f x x x x ⎛⎫⎛⎫'''-+ ⎪ ⎪⎝⎭⎝⎭ B.231121f f x x x x ⎛⎫⎛⎫'''+ ⎪ ⎪⎝⎭⎝⎭ C.231121f f x x x x ⎛⎫⎛⎫'''--⎪ ⎪⎝⎭⎝⎭D.231121f f x x x x ⎛⎫⎛⎫'''-⎪ ⎪⎝⎭⎝⎭（1306）已知函数()f x 在点1x =处连续，且21()1lim 12x f x x →=-，则曲线()f x 在点()1,()f x 处切线方程为（） A.1y x =-B.22y x =-C.33y x =-D.44y x =-（1309）设函数由参数方程2211x t y t ⎧=+⎨=-⎩所确定，则221d d t yx == ．（二）中值定理及导数的应用（0423）甲、乙二城位于一直线形河流的同一侧，甲城位于岸边，乙城离河岸40公里，乙城在河岸的垂足与甲城相距50公里，两城计划在河岸上合建一个污水处理厂，已知从污水处理厂到甲乙二城铺设排污管道的费用分别为每公里500、700元．问污水处理厂建在何处，才能使铺设排污管道的费用最省？（0507）02limsin x x x e e xx x-→--=- ．（0508）函数x x f ln )(=在区间[]1,e 上满足拉格郎日中值定理的=ξ ．（0521）证明方程：0133=+-x x 在[]1,1-上有且仅有一根．（0603）下列函数在[]1,1-上满足罗尔定理条件的是（） A.xe y =B.1y x =+C.21x y -=D.xy 11-= （0621）证明：当2x ≤时，332x x -≤．（0703）设函数()(1)(2)(3)f x x x x x =---，则方程()0f x '=的实根个数为（） A.1B.2C.3D.4（0713）求极限01lim tan x x e x x x→--．（0722）设函数9)(23-++=cx bx ax x f 具有如下性质：（1）在点1-=x 的左侧临近单调减少；（2）在点1-=x 的右侧临近单调增加；（3）其图形在点(1,2)的两侧凹凸性发生改变．试确定a ，b ，c 的值．（0724）求证：当0>x 时，22(1)ln (1)x x x -⋅≥-．（0809）已知曲线543223++-=x x x y ，则其拐点为．（0821）求曲线1y x=（0x >）的切线，使其在两坐标轴上的截距之和最小，并求此最小值．（0823）设函数)(x f 在闭区间[]0,2a （0a >）上连续，且)()2()0(a f a f f ≠=，证明：在开区间(0,)a 上至少存在一点ξ，使得()()f f a ξξ=+．（0824）对任意实数x ，证明不等式：(1)1xx e -⋅≤．（0904）曲线221(1)x y x +=-的渐近线的条数为（）A.1B.2C.3D.4（0913）求极限30lim sin x x x x→-．（0921）已知函数13)(3+-=x x x f ，试求：（1）函数)(x f 的单调区间与极值；（2）曲线)(x f y =的凹凸区间与拐点；（3）函数)(x f 在闭区间[2,3]-上的最大值与最小值．（0924）证明：当12x <<时，24ln 23x x x x >+-．（1002）曲线223456x x y x x -+=-+的渐近线共有 ( )A.1条B.2条C.3条D.4条（1006）设3()3f x x x =-，则在区间(0,1)内 ( ) A.函数()f x 单调增加且其图形是凹的 B.函数()f x 单调增加且其图形是凸的 C.函数()f x 单调减少且其图形是凹的 D.函数()f x 单调减少且其图形是凸的（1013）求极限2|011lim tan x x x x →⎛⎫-⎪⎝⎭．（1021）证明：当1x >时，121122x e x ->+．（1103）若点(1,2)-是曲线23bx ax y -=的拐点，则（） A.3,1==b aB.1,3-=-=b aC.3,1-=-=b aD.6,4==b a（1113）求极限()()22limln 1xx x eex -→-+．（1121）证明：方程()2ln 12x x ⋅+=有且仅有一个小于2的正实根．（1122）证明：当0>x 时，x x201120102011≥+．（1203）设232152)(x x x f -=，则函数)(x f ( ) A.只有一个最大值 B.只有一个极小值 C.既有极大值又有极小值D.没有极值（1213）求极限()2302cos 2lim ln 1x x x x x →+-+．（1223）证明：当10<<x 时，361arcsin x x x +>．（1302）曲线22232x xy x x +=-+的渐近线共有（）A.1条B.2条C.3条D.4条（1313）求极限01lim ln (1)x x e x x →⎡⎤-⎢⎥+⎣⎦．（1323）证明：当1x >时，2(1ln )21x x +<-．三、一元函数积分学（一）不定积分（0410）求不定积分3x = ．（0416）设)(x f 的一个原函数为xe x，计算(2)d x f x x '⎰．（0503）若()d ()f x x F x C =+⎰，则sin (cos )d x f x x =⎰（）A.C x F +)(sinB.C x F +-)(sinC.C F +(cos)D.C x F +-)(cos（0515）计算3tan sec d x x x ⎰．（0522）设函数)(x f y =的图形上有一拐点(2,4)P ，在拐点处的切线斜率为3-，又知该函数的二阶导数6y x a ''=+，求)(x f ．（0604）已知2()d x f x x e C =+⎰，则()d f x x '-=⎰（）A.C ex+-22B.C e x +-221 C.C e x +--22 D.C e x +--221（0615）计算x ．（0622）已知曲线)(x f y =过原点且在点),(y x 处的切线斜率等于y x +2，求此曲线方程．（0704）设函数)(x f 的一个原函数为x 2sin ，则(2)d f x x '=⎰（）A.C x +4cosB.C x +4cos 21C.C x +4cos 2D.C x +4sin（0715）求不定积分2d x x e x -⎰．（0810）设函数)(x f 的导数为x cos ，且21)0(=f ，则不定积分()d f x x =⎰ ．（0815）求不定积分3d 1x x x +⎰．（0905）设()ln (31)F x x =+是函数)(x f 的一个原函数，则(21)d f x x '+=⎰（）A.C x ++461B.C x ++463C.C x ++8121D.C x ++8123（0915）求不定积分x ⎰．（1015）求不定积分arctan d x x x ⎰．（1115）设)(x f 的一个原函数为x x sin 2，求不定积分()d f x x x⎰．（1215）求不定积分sin 2d x x x ⎰．（1315）求不定积分sin 2d x x x ⎰．（二）定积分（0404）2228R y x =+设所围的面积为S ，则0x ⎰的值为（）A.SB.4S C.2S D.S 2（0421）证明：0(sin )d (sin )d 2x f x x f x x πππ=⎰⎰，并利用此式求20sin d 1cos xxx xπ+⎰．（0509）1211d 1x x x π-+=+⎰．（0516）计算10arctan d x x ⎰．（0609）设)(x f 在[]0,1上有连续的导数且(1)2f =，10()d 3f x x =⎰，则1()d x f x x '=⎰ ．（0616）计算22cos d x x x π⎰．（0709）定积分)231cos d x x x -+⎰的值为．（0716）计算定积分x ．（0811）定积分1212sin d 1xx x -++⎰的值为．（0816）求定积分10d x ⎰．（0916）求定积分：210⎰．（1009）定积分31211d 1x x x -++⎰的值为．（1016）计算定积分40x ⎰．（1111）定积分()32221sin d xx x ππ-+⋅⎰的值为____________．（1116）计算定积分3⎰　．（1216）计算定积分21⎰．（1316）计算定积分20⎰（1324）设函数()f x 在[,]a b 上连续，证明：[]2()d ()()d a b b aaf x x f x f a b x x +=++-⎰⎰．（三）变限积分与广义积分（0417）计算广义积分2+∞⎰（0422）设函数)(x f 可导，且满足方程20()d 1()x t f t t x f x =++⎰，求)(x f ．（0705）设221()sin d x f x t t =⎰，则()f x '=（）A.4sin x B.2sin 2x xC.2cos 2x xD.4sin 2x x（0803）设函数)(x f 122sin d xt t t =⎰，则()f x '等于（）A.x x 2sin 42B.x x 2sin 82C.x x 2sin 42-D.x x 2sin 82-（0908）设函数20()d x t x te t ϕ=⎰，则()x ϕ'＝．（1003）设函数22()cos d t xx e t t Φ=⎰，则函数()x Φ的导数()x 'Φ等于 ( )A.222cos x xe x B.222cos x xe x - C.2cos xxe x - D.22cos x e x - （1108）设函数2()ln (1)d x x t t Φ=+⎰　，则=Φ'')1(____________．（1211）设反常积分1d 2x ae x +∞-=⎰，则常数=a ______．（1222）已知定义在(),-∞+∞上的可导函数)(x f 满足方程31()4()d 3xx f x f t t x -=-⎰，试求：（1）函数()f x 的表达式；（2）函数)(x f 的单调区间与极值；（3）曲线()y f x =的凹凸区间与拐点．（1224）设0()d 0()(0)0x g t t x f x g x ⎧≠⎪=⎨⎪=⎩⎰，其中函数)(x g 在(,)-∞+∞上连续，且3cos 1)(lim 0=-→xx g x ．证明：函数)(x f 在0=x 处可导，且1(0)2f '=．（1322）已知251320()95d x F x t t t ⎛⎫=- ⎪⎝⎭⎰是()f x 的一个原函数，求曲线()y f x =的凹凸区间、拐点．（四）定积分的几何应用（0523）已知曲边三角形由x y 22=、0=x 、1=y 所围成，求：（1）曲边三角形的面积；（2）曲边三角形绕x 轴旋转一周的旋转体体积．（0623）已知一平面图形由抛物线2x y =、82+-=x y 围成．（1）求此平面图形的面积；（2）求此平面图形绕y 轴旋转一周所得的旋转体的体积．（0721）设平面图形由曲线21x y -=（0≥x ）及两坐标轴围成．（1）求该平面图形绕x 轴旋转所形成的旋转体的体积；（2）求常数a 的值，使直线a y =将该平面图形分成面积相等的两部分．（0822）设平面图形由曲线2x y =，22x y =与直线1=x 所围成．（1）求该平面图形绕x 轴旋转一周所得的旋转体的体积；（2）求常数a ，使直线a x =将该平面图形分成面积相等的两部分．（0922）设1D 是由抛物线22x y =和直线x a =，0y =所围成的平面封闭区域，2D 是由抛物线22x y =和直线x a =，2x =及0=y 所围成的平面封闭区域，其中20<<a ．试求：（1）1D 绕y 轴旋转所成的旋转体的体积1V ，以及2D 绕x 轴旋转所成的旋转体的体积2V ；（2）求常数a 的值，使得1D 的面积与2D 的面积相等．（1023）设由抛物线2y x =（0x ≥），直线2y a =（01a <<）与y 轴所围成的平面图形绕x 轴旋转一周所形成的旋转体的体积记为1()V a ，由抛物线2y x =（0x ≥），直线2y a =（01a <<）与直线1x =所围成的平面图形绕x 轴旋转一周所形成的旋转体的体积记为2()V a ，另12()()()V a V a V a =+，试求常数a 的值，使()V a 取得最小值．（1024）设函数()f x 满足方程()()2xf x f x e '+=，且(0)2f =，记由曲线'()()f x y f x =与直线1y =，x t =（0t >）及y 轴所围平面图形的面积为()A t ，试求lim ()t A t →+∞．（1124）设函数)(x f 满足微分方程()2()(1)x f x f x a x '-=-+（其中a 为正常数），且1)1(=f ，由曲线()y f x =（1x ≤）与直线1x =，0y =所围成的平面图形记为D ．已知D 的面积为32．（1）求函数)(x f 的表达式；（2）求平面图形D 绕x 轴旋转一周所形成的旋转体的体积x V ；（3）求平面图形D 绕y 轴旋转一周所形成的旋转体的体积y V ．（1221）在抛物线2y x =（0x >）上求一点P ，使该抛物线与其在点P 处的切线及x 轴所围成的平面图形的面积为32，并求该平面图形绕x 轴旋转一周所形成的旋转体的体积．（1321）设平面图形D 是由曲线x =y =1y =所围成，试求：（1）平面图形D 的面积；（2）平面图形D 绕x 轴旋转一周所形成的旋转体的体积．四、向量代数与空间解析几何（一）向量代数（0510）设向量{}3,4,2=-a 、{}2,1,k =b ；a 、b 互相垂直，则=k ．（0610）设1=a ，⊥a b ，则()⋅+=a a b ．（0710）已知a 、b 均为单位向量，且12⋅=a b ，则以a 、b 为邻边的平行四边形面积为．（0804）设向量(1,2,3)=a ，(3,2,4)=b ，则⨯a b 等于（）A.(2,5,4)B.(2,5,4)--C.(2,5,4)-D.(2,5,4)--（0909）已知向量{}1,0,1=-a ，{}1,2,1=-b ，则+a b 与a 的夹角为．（1010）设{}1,2,3=a ，{}2,5,k=b ，若a 与b 垂直，则常数k = ．（1109）若1=a ，4=b ，2⋅=a b ，则⨯=a b ____________．（1210）设向量a 、b 互相垂直，且3=a ，2=b ，则2+=a b ________．（1308）已知空间三点(1,1,1)A ，(2,3,4)B ，(3,4,5)C ，则ABC ∆的面积为．（二）平面与直线（0518）求过点(3,1,2)A -且通过直线L ：43521x y z-+==的平面方程．（0619）求过点(3,1,2)M -且与二平面07=-+-z y x 、0634=-+-z y x 都平行的直线方程．（0719）求过点(1,2,3)且垂直于直线20210x y z x y z +++=⎧⎨-++=⎩的平面方程．（0817）设平面∏经过点(2,0,0)A ，(0,3,0)B ，(0,0,5)C ，求经过点(1,2,1)P 且与平面∏垂直的直线方程．（0917）求通过直线12213-=-=z y x 且垂直于平面02=+++z y x 的平面方程．（1017）求通过点(1,1,1)，且与直线23253x ty t z t =+⎧⎪=+⎨⎪=+⎩垂直，又与平面250x z --=平行的直线的方程．（1117）求通过x 轴与直线132zy x ==的平面方程．（1217）已知平面∏通过(1,2,3)M 与x 轴，求通过(1,1,1)N 且与平面∏平行，又与x 轴垂直的直线方程．（1318）已知直线10330x y z x y z -+-=⎧⎨--+=⎩在平面∏上，又知直线23132x ty t z t=-⎧⎪=+⎨⎪=+⎩与平面∏平行，求平面∏的方程．五、多元函数微积分（一）多元函数微分学（0418）设(,)z f x y xy =-，且具有二阶连续的偏导数，求x z ∂∂、yx z∂∂∂2．（0505）设yxy x u arctan),(=，(,)v x y =，则下列等式成立的是（）A.yv x u ∂∂=∂∂ B.xvx u ∂∂=∂∂ C.x v y u ∂∂=∂∂ D.y v y u ∂∂=∂∂ （0517）已知函数2(sin ,)z f x y =，其中),(v u f 有二阶连续偏导数，求x z ∂∂、yx z∂∂∂2．（0611）设x e u xysin =，=∂∂xu．（0620）设2(,)z x f x xy =⋅其中(,)f u v 的二阶偏导数存在，求y z ∂∂、xy z∂∂∂2．（0711）设yxz =，则全微分d z = ．（0717）设(23,)z f x y xy =+其中f 具有二阶连续偏导数，求yx z∂∂∂2．（0805）函数xyz ln =在点(2,2)处的全微分d z 为（）A.11d d 22x y -+B.11d d 22x y +C.11d d 22x y -D.11d d 22x y --（0818）设函数,y z f x y x ⎛⎫=+ ⎪⎝⎭，其中)(x f 具有二阶连续偏导数，求y x z ∂∂∂2．（0910）设函数(,)z z x y =由方程12=+yz xz 所确定，则xz∂∂＝．（0919）设函数(sin ,)z f x xy =，其中)(x f 具有二阶连续偏导数，求yx z∂∂∂2．（1011）设函数z =，则10d x y z=== ．（1018）设()2,xz y f xy e =⋅，其中函数f 具有二阶连续偏导数，求2zx y∂∂∂．（1104）设),(y x f z =为由方程8333=+-x yz z 所确定的函数，则=∂∂==00y x yz （）A.21-B.21C.2-D.2（1118）设)(y xyxf z ，=，其中函数f 具有二阶连续偏导数，求y x z ∂∂∂2．（1204）设3ln 2z x y=+在点()1,1处的全微分为 ( )A.d 3d x y -B.d 3d x y +C.1d 3d 2x y +D.1d 3d 2x y -（1218）设函数22(,)()z f x xy x y ϕ=++，其中函数f 具有二阶连续偏导数，函数()x ϕ具有二阶连续导数，求yx z∂∂∂2．（1314）设函数(,)z z x y =由方程3331z xy z +-=所确定，求d z 及22zx∂∂．（1317）设()223,x yz fx e+=，其中函数f 具有二阶连续偏导数，求2zy x∂∂∂．（二）二重积分（0411）交换二次积分的次序2120d (,)d x x x f x y y -=⎰⎰．（0419）计算二重积分sin d d Dyx y y ⎰⎰，其中D 由曲线x y =及x y =2所围成．（0504）设区域D 是xoy 平面上以点(1,1)A 、(1,1)B -、(1,1)C --为顶点的三角形区域，区域1D 是D 在第一象限的部分，则(cos sin )d d Dxy x y x y +=⎰⎰（）A.⎰⎰1)sin (cos 2D dxdy y xB.⎰⎰12D xydxdyC.⎰⎰+1)sin cos (4D dxdy y x xyD. 0（0511）交换二次积分的次序11d (,)d x x f x y y -+=⎰；（0524）设)(x f 为连续函数，且1)2(=f ，1()d ()d uuyF u y f x x =⎰⎰（1u >）．（1）交换)(u F 的积分次序；（2）求(2)F '．（0606）设对一切x 有(,)(,)f x y f x y -=-，22{(,)|1,0}D x y x y y =+≤≥，=1D 22{(,)|1,0,0}x y x y x y +≤≥≥，则(,)d d Df x y x y =⎰⎰（）A. 0B.1(,)d d D f x y x y ⎰⎰C.21(,)d d D f x y x y ⎰⎰D.41(,)d d D f x y x y ⎰⎰（0612）D 为以点(0,0)O 、(1,0)A 、(0,2)B 为顶点的三角形区域，d d Dx y =⎰⎰ ．（0624）设⎪⎩⎪⎨⎧=≠=⎰⎰00)(1)(t a t dxdy x f t t g tD ，其中t D 是由t x =、t y =以及坐标轴围成的正方形区域，函数)(x f 连续．（1）求a 的值使得)(t g连续；（2）求)('t g ．（0720）计算二重积分d Dx y ，其中{}22(,)|2,0D x y x y x y =+≤≥．（0723）设0>>a b ，证明：()232d ()d ()d b b b x y xx a ayay f x e x ee f x x ++⋅=-⎰⎰⎰．（0819）计算二重积分2d d Dx x y ⎰⎰，其中D 是由曲线xy 1=，直线y x =，2x =及0=y 所围成的平面区域．（0918）计算二重积分d Dy σ⎰⎰，其中22{(,)02,2,2}D x y x x y x y =≤≤≤≤+≥．（1005）二次积分111d (,)d y y f x y x +⎰⎰交换积分次序后得 ( )A.1101d (,)d x x f x y y +⎰⎰B.2110d (,)d x x f x y y -⎰⎰C.2111d (,)d x x f x y y -⎰⎰D.2111(,)d x dx f x y y -⎰⎰（1019）计算d d Dx x y ⎰⎰，其中D 是由曲线x =y x =及x 轴所围成的闭区域．（1105）若(,)d d Df x y x y ⎰⎰可转化为二次积分1201d (,)d y y f x y x +⎰⎰ ，则积分域D 可表示为（） A.{}(,)01,11x y x x y ≤≤-≤≤ B.{}(,)12,11x y x x y ≤≤-≤≤C.{}(,)01,10x y x x y ≤≤-≤≤D.{}(,)12,01x y x y x ≤≤≤≤-（1119）计算二重积分d d Dy x y ⎰⎰，其中D 是由曲线y =直线x y -=及y 轴所围成的平面闭区域．（1205）二次积分dx y x f dy y),(11⎰⎰ 在极坐标系下可化为（）A.sec 40d (cos ,sin )d f πθθρθρθρ⎰⎰ B.sec 40d (cos ,sin )d f πθθρθρθρρ⎰⎰C.sec 24d (cos ,sin )d f πθπθρθρθρ⎰⎰D .sec 24d (cos ,sin )d f πθπθρθρθρρ⎰⎰ （1220）计算二重积分d d Dy x y ⎰⎰，其中D 是由曲线y =2xy =及x 轴所围成的平面闭区域．（1320）计算二重积分d d Dx x y ⎰⎰，其中D 是由曲线y =0x >）与三条直线y x =，3x =，0y =所围成的平面闭区域．六、无穷级数（一）数项级数（0506）正项级数（1）∑∞=1n n u 、（2）∑∞=13n n u ，则下列说法正确的是（） A.若（1）发散、则（2）必发散 B.若（2）收敛、则（1）必收敛C.若（1）发散、则（2）不确定D.（1）、（2）敛散性相同（0605）设∑∞=1n nu为正项级数，如下说法正确的是（）A.若0lim 0=→n n u ，则∑∞=1n nu必收敛 B.若l u u nn n =+∞→1lim )0(∞≤≤l ，则∑∞=1n n u 必收敛C.若∑∞=1n nu收敛，则∑∞=12n nu必定收敛D.若∑∞=-1)1(n n nu 收敛，则∑∞=1n n u 必定收敛（0706）下列级数收敛的是（）A.∑∞=122n nnB.∑∞=+11n n n C.∑∞=-+1)1(1n nnD.∑∞=-1)1(n nn（0906）设α为非零常数，则数项级数∑∞=+12n n n α（）A.条件收敛B.绝对收敛C.发散D.敛散性与α有关（1004）下列级数收敛的是（）A.11n nn ∞=+∑B.2121n n n n ∞=++∑C.nn ∞= D.212n n n ∞=∑（1206）下列级数中条件收敛的是（）A.1(1)21nn nn ∞=-+∑B.13(1)2nnn ∞=⎛⎫- ⎪⎝⎭∑C.21(1)nn n ∞=-∑D.1nn ∞=（1305）下列级数中收敛的是（）A.211n n n∞=+∑ B.11nn n n ∞=⎛⎫ ⎪+⎝⎭∑C.1!2n n n ∞=∑D.1n ∞= （二）幂级数（0412）幂级数∑∞=-12)1(n nnx 的收敛区间为．（0420）把函数21)(+=x x f 展开为2-x 的幂级数，并写出它的收敛区间．（0512）幂级数1(21)nn n x∞=-∑的收敛区间为．（0519）把函数222)(x x x x f --=展开为x 的幂级数，并写出它的收敛区间．（0618）将函数()ln (1)f x x x =+展开为x 的幂函数（要求指出收敛区间）．（0812）幂函数12n nn x n ∞=⋅∑的收敛域为．（0911）若幂函数21n nn a x n∞=∑（0a >）的收敛半径为21，则常数=a ．（1012）幂级数0(1)n nn x n ∞=-∑的收敛域为．（1106）若x x f +=21)(的幂级数展开式为0()nn n f x a x ∞==∑（22x -<<），则系数=n a ( )A.n 21B.121+n C.(1)2nn -D.1(1)2nn +-（1112）幂级数0nn ∞=的收敛域为_ _ _________．（1212）幂级数1(1)(3)3nn nn x n ∞=--⋅∑的收敛域为____________．（1312）幂级数1n nn ∞=的收敛域为．七、常微分方程（一）一阶微分方程（0520）求微分方程0'=-+xe y xy 满足1x ye ==的特解．（0617）求微分方程22x y xy y '=-的通解．（0718）求微分方程22007xy y x '-=满足初始条件12008x y==的特解．（0820）求微分方程22xy y x '=+的通解．（0912）微分方程2(1)d (2)d 0x y x y x y +--=的通解为．（1311）微分方程d d y x y x x+=的通解为．（二）二阶线性微分方程（0406）微分方程232xy y y xe '''-+=的特解*y 的形式应为（）A.xAxe 2B.xe B Ax 2)(+C.xeAx 22D.xeB Ax x 2)(+（0712）设x xe C eC y 3221+=为某二阶常系数齐次线性微分方程的通解，则该微分方程为．（0806）微分方程321y y y '''++=的通解为（）A.1221++=--x xe c e c yB.21221++=--x xe c ec yC.1221++=-xxec e c yD.21221++=-xxec e c y （0920）求微分方程y y x ''-=的通解．（1020）已知函数xy e =和2xy e-=是二阶常系数齐次线性微分方程0y py qy '''++=的两个解，试确定常数p 、q 的值，并求微分方程xy py qy e '''++=的通解．（1120）已知函数(1)xy x e =+⋅是一阶线性微分方程2()y y f x '+=的解，求二阶常系数线性微分方程)(23x f y y y =+'+''的通解．（1219）已知函数)(x f 的一个原函数为xxe ，求微分方程)(44x f y y y =+'+''的通解．（1319）已知函数()y f x =是一阶微分方程d d yy x=满足初始条件(0)1y =的特解，求二阶常系数非齐次线性微分方程32()y y y f x '''-+=的通解．时间排序与参考答案2004年高等数学真题参考答案1、A ．2、B ．3、C ．4、B ．5、A ．6、D ．7、1-e ． 8、32241-+==-z y x ． 9、!n ． 10、C x +4arcsin 41． 11、12201d (,)d d (,)d y y f x y x y f x y x -+⎰⎰⎰．12、()3,1-．13、解：间断点为πk x =（Z k ∈），当0=x 时，1sin lim)(lim 00==→→xxx f x x ，为可去间断点；当πk x =（0≠k ，Z k ∈）时，∞=→xxx sin lim0，为第二类间断点．14、解：原式0430(tan sin )d tan sin limlim312xx x t t tx xx x →→--==⎰233001tan (1cos )12lim lim 121224x x x x x x x x →→⋅-===． 15、解：0=x 代入原方程得1)0(=y ，对原方程求导得0''=--y xe e y yy，对上式求导并将0=x 、1=y 代入，解得：22''e y =．16、解：因为)(x f 的一个原函数为x e x，所以2')1()(x e x x e x f xx -=⎪⎪⎭⎫ ⎝⎛=，原式11(2)d(2)d (2)22xf x x x f x '==⎰⎰11(2)(2)d 22x f x f x x =-⎰ 222211(21)1(2)(2)d(2)24884x x xx x e e x x f x f x x C e C x x x--=-=-+=+⎰． 17211122d d 22arctan (1)12t tt tt t t π+∞∞+∞+===++⎰．18、解：12zf f y x∂''=+⋅∂； []21112221221112222(1)(1)()zf f x f y f f x f x y f xy f f x y∂''''''''''''''''=⋅-+⋅++⋅-+⋅=-+-⋅+⋅+∂∂．19、解：原式21100sin sin d d d d (1)sin d y y Dyy x y y x y y y y y ===-⎰⎰⎰⎰⎰ 1100(1)cos cos d 1sin1y y y y =--=-⎰．20、解：01111(2)()(1)24244414n n nn x f x x x ∞=-==⋅=--+-+∑)62(<<-x ． 21、证：00(sin )d ()[sin ()]d ()(sin )d t xx f x xt f t t t f t I t πππππππ=-=---=-⎰⎰⎰(sin )d (sin )d (sin )d f x x x f x x f x x I πππππ=-=-⎰⎰⎰解得： 0(sin )d (sin )d 2f x x f x x I x πππ==⎰⎰，原命题证毕．222000sin sin d d arctan (cos )1cos 21cos 24x x x x x x x x ππππππ⋅==-=++⎰⎰． 22、解：等式两边求导得()2()x f x x f x '=+，即()()2f x x f x x '-=-，且(0)1f =-，x p -=，x q 2-=，而2()d 2x x xe e --⎰=，由公式求得通解：222222()2d 2x x x f x e xq x C C e -⎡⎤⎛⎫=-+=+⎢⎥ ⎪ ⎪⎢⎥⎝⎭⎣⎦⎰，将初始条件(0)1f =-代入通解，解得：3-=C ，故22()23x f x e =-．23、解：设污水厂建在河岸离甲城x 公里处，则()500M x x =+500≤≤x ），由150070002M '=+⨯=解得：650050-=x （公里），唯一驻点，即为所求．2005年高等数学真题参考答案1、A ．2、C ．3、D ．4、A ．5、A ．6、C ．7、2． 8、1-e ． 9、2π． 10、5． 11、11d (,)d y y f x y x -⎰⎰．12、(1,1)-．13、解：因为)(x F 在0=x 处连续，所以)0()(lim 0F x F x =→，'00()2sin ()(0)lim ()limlim 2(0)28x x x f x x f x f F x f x x→→→+-==+=+=，解得：a F =)0(，故8=a ．14、解：d d cos cos sin d d d sin d yy t t t t t t x x t t-+===--，22d ()csc d (cos )y t t x t '-=='．15、解：原式22tan tan sec d (sec1)d(sec )x x x xx x =⋅-⎰⎰积进去231sec d(sec )d(sec )sec sec 3x x x x x C =-=-+⎰⎰．16、解：原式211120002d 1d(1)arctan 1421x x x x x x x π+=--++⎰⎰积进去 ()12011ln 1ln 24242x ππ⎡⎤=-+=-⎣⎦．17、解：1cos zx f x∂'=⋅∂，()21212cos 22cos z x f y y x f x y ∂''''=⋅⋅=⋅∂∂． 18、解：直线L 的方向向量{}5,2,1=s ，过点()4,3,0B -，{}1,4,2AB =-；所求平面的法向量{}5218,9,22142AB =⨯==---ij kn s ，点法式为8(3)9(1)22(2)0x y z ----+=，即592298=--z y x ．19、解：2222101111(1)()13216313212n nn n x x x x f x x x x x x ∞+=⎡⎤-⎛⎫=+=⋅+⋅=+⋅ ⎪⎢⎥+--⎝⎭⎣⎦+∑，收敛域为：11<<-x ．20、解：1x e y y x x '+⋅=，即1p x=，x e q x =，而1d 1x x e x -⎰=；故通解为1d xx e e C y x x C x x x ⎛⎫+=+= ⎪⎝⎭⎰．把初始条件1x y e ==解得：0=C ；故所求特解为：xe y x=．21、证：令13)(3+-=x x x f ，[]1,1x ∈-，且(1)30f -=>，(1)10f =-<，(1)(1)0f f -⋅<；由连续函数零点定理知：)(x f 在(1,1)-内至少有一实根；对于()1,1x ∈-恒有()22()33310f x x x '=-=-<，即)(x f 在(1,1)-内单调递减，故方程0133=+-x x 在[]1,1-上有且仅有一根；原命题获证．22、解：设所求函数为)(x f y =，则有4)2(=f ，(2)3f '=-，(2)0f ''=；由()6f x x a ''=+和(2)0f ''=解得：12-=a ，即()612f x x ''=-，故21()312f x x x C '=-+，由(2)3f '=-解得：91=C ，故22396C x x x y ++-=，由(2)4f =解得：22=C ；所求函数为：29623++-=x x x y ．23、解：（1）112300111d 266S y y y ===⎰；（如图1所示）（2）()()112222012d 4x V x x x xπππ=-=-=⎰．24、解：积分区域D 为：u y ≤≤1，u x y ≤≤；（1）111()()d d ()d (1)()d u xuDF u f x x f x y x f x x σ===-⎰⎰⎰⎰⎰；（2）()(1)()F u u f u '=-，(2)(21)(2)(2)1F f f '=-==．2006年高等数学真题参考答案1、C ．2、B ．3、C ．4、C ．5、C ．6、A ．7、2． 8、)(0x f ． 9、1-． 10、1． 11、(sin cos )xye y x x +． 12、1．13、解：原式322131lim 21341==--→x xx ．图114、解：2211d 12d 21t t y y t t t x x t-'+==='+，2222d 1d d 122d 41ty x y t t x x t t '⎛⎫ ⎪+⎝⎭==='+． 15、解：原式322ln )(1ln )3x x C =+=++．16、解：原式()2222220d(sin )sin 2sin d x x x xx x πππ=-⎰⎰积进去222220sin 2sin d 2d(cos )4x xx x xx x ππππ-+⎰⎰积进去导出来2222002cos 2cos d 244x x x x ππππ=+-=-⎰．17、解：方程变形为2y y y x x ⎛⎫'=- ⎪⎝⎭，即得到了形如d d y y f x x ⎛⎫= ⎪⎝⎭齐次方程；令y u x =，则d d d d y u u x x x =+，代入得：2d d u x u x =-，分离变量得：211d d u x u x-=；两边积分，得：211d d u x u x -=⎰⎰，1ln x C u=+，故ln x y x C =+． 18、解：令()ln (1)g x x =+，则(0)0g =；由于01()(1)1n n n g x x x ∞='==-+∑（(]1,1x ∈-），所以01(1)((1))d x n n n g x n x g t t ∞+='=+=-∑⎰（(]1,1x ∈-），故20(1)()1n n n f x x n ∞+=-=+∑，收敛域为：11x -<≤．19、解：由题意知：{}11,1,1=-n ，{}24,3,1=-n ；{}12311232,3,1431=⨯=-=++=-i j ks n n i j k ，故所求直线方程的对称式方程为：123123+=-=-z y x ．20、解：22z x f x∂'=∂，2'2'''''3''2''22122221222(2)22z x f x f x f y x f x f x y f y x ∂=+⋅+⋅=++∂∂． 21、证：令33)(x x x f -=，[]2,2x ∈-，由2()330f x x '=-=解得驻点：1±=x ，比较以下函数值的大小：(1)2f -=-，(1)2f =，(2)2f =-，(2)2f -=；所以2min -=f ，2m ax =f ，故2)(2≤≤-x f ，即332x x -≤，原命题获证．22、解：0)0(=y ，2y x y '=+，通解为：xCe x y +--=)22(；将0)0(=y 代入通解解得：2=C ，故所求特解为：xe x y 222+--=．23、解：（1）()2222648d 3S x x x -=--=⎰；（2）224804d d 16y V y y πππ=+=⎰⎰．24、解：()d d d ()d ()d tt tt D f x x y x f x y t f x x ==⎰⎰⎰⎰⎰，0()d 0()0t f x x t g t a t ⎧≠⎪=⎨⎪=⎩⎰；（1）00lim ()lim()d 0t t t g t f x x →→==⎰，由)(t g 的连续性可知：0)(lim )0(0===→t g g a t ；（2）当0≠t 时，()()g t f t '=，当0=t 时，0000()d ()(0)(0)limlim lim ()(0)hh h h f x x g h g g f h f h h→→→-'====⎰；综上，()()g t f t '=．2007年高等数学真题参考答案1、B ．2、C ．3、C ．4、A ．5、D ．6、D ．7、2ln ． 8、1． 9、π2． 10、23． 11、21d d xx y y y-． 12、06'5''=+-y y y ． 13、解：212lim 21lim 1lim tan 1lim00200==-=--=--→→→→x x x x x x x x e x e x x e x x x e ． 14、解：当0=x 时，0=y ；。

2004年江苏省专转本真题讲解

2004年江苏省普通高校“专转本”统一考试Part I Reading Comprehension （共20小题，每小题2分，共40分）Directions: In this part there are four passages. Each passage is followed by four comprehension questions. Read the passage and answer the questions. Then mark your answer on the Answer Sheet.Passage 1Questions 1 to 5 are based on the following passage:Some years ago the captain of a ship was very interested in medicine. He always took medicine books to sea and liked to talk about different diseases.One day a lazy sailor on his ship pretended to be ill. He lay on his bunk (铺) and groaned as if he were very sick. The captain came to see him and was very pleased to have a patient to look after. He told the man to rest for a few days and made the other sailors do his work. Three days later another sailor pretended that he had something wrong with his chest. Once more the captain looked in his medical books and told “sick” man to have a rest.The other sailors were very angry because they had more work to do. The patients had the best food and laughed at their friends when the captain was not looking. At last the mate (船长副手) decided to cure the “sick” men. He mixed up some soap, soot (烟灰), glue (胶水) and other unpleasant things. Then he obtained permission from the captain to give his medicine to the “sick”men. When they tasted the medicine, they really did feel ill. It was so horrible that one of the patients jumped out of hi bunk, ran up on desk and climbed the highest mast on the ship. He did not want any more medicine.The mate told both of the men that they must take the medicine every half an hour, night and day. This soon cured them. They both said they felt better and wanted to start word again. The captain realized that the men tried to deceive him so he made them work very hard for the rest of the voyage.1. The first sailor pretended to be ill because he wanted to .A. test the captain’s knowledge of medicineB. be free from workC. have the best food on the shipD. play a joke on his friends2. When the captain knew a sailor was ill, he .A. didn’t care muchB. sent for a doctorC. looked after him and told him to have a restD. gave him some medicine3. The patients felt better quickly because .A. they had been given proper medicineB. they learned that the captain had found out the truthC. they were laughed at by their friendsD. the medicine the mate gave was horrible4. When the captain knew he had been deceived, he .A. told them not to do so againB. lost his temperC. made them work harderD. fired them5. Which of the following best summarizes the passage?A. A sudden Cure.B. Two Patients.C. Captain and Sailors.D. A Difficult V oyage. Passage 2Questions 6 to 10 are based on the following passage:When aluminum was first produced about a hundred and fifty years ago, it was so difficult to separate form the ores in which it was found that its price was higher than that of gold. The price remained high until a new process was discovered for refining the metal with the aid of electricity approximately three quarters of a century later. The new method was so much cheaper that aluminum because practical for many purposes, one of which was making pots and pans.Aluminum is lightweight, rustproof and easily shaped into different forms. By mixing it with other metals, scientists have been able to produce a variety of alloys, some of which have the strength of steelbut weigh only one third as much.Today, the uses of aluminum are innumerable. Perhaps its most important use is in transportation. Aluminum is found in the engine of automobiles, in the hulls of boats. It is also used in many parts of airplanes. In fact, the huge “airbus” planes would probably never have been produced if aluminum did not exist. By making vehicles lighter in weight aluminum has greatly reduced the amount of fuel needed to move them, Aluminum is also being used extensively in the building industry in some countries.Since aluminum is such a versatile (多用的) metal, it is fortunate that bauxite (铝土矿), which is one of its chief sources, is also one of the earth’s most plentiful substances. As the source of aluminum is almost inexhaustible, we can expect that more and more uses will be found for this versatile metal.6. The price of aluminum was sharply reduced when people discovered a new refining process with the aid of .A. windB. solar energyC. hydraulic powerD. electricity7. Aluminum is .A. lightweight, rustproof but not easily shaped into different formsB. heavyweight, rustproof and easily shaped into different formsC. lightweight, rustproof and easily shaped into different formsD. lightweight and easily shaped into different forms but it is easy to become rusty8. Which of the following is NOT true?A. Aluminum is widely used in transportation.B. Aluminum is also used in many parts of airplanes.C. Aluminum is being used extensively in the building industry.D. Aluminum is not used in its pure form.9. Aluminum is found on earth mostly in the form of .A. pure metalB. bauxiteC. goldD. liquid10. What is the passage talking about?A. The features of aluminum and its functions.B. The process of aluminum.C. The discovery of aluminum.D. The promising future of aluminum.Passage 3Questions 11 to 15 are based on the following passage:The idea of a special day to honor mothers was first put forward in America in 1907. two years later a woman, Mrs. John Bruce Dodd, in the state of Washington proposed a similar day to honor the head of the family—the father. Her mother died when she was very young, and her father brought her up. She loved her father very much.In response to Mrs. Dodd’s idea that same year—1909, the state governor of Washington proclaimed (宣布) the third Sunday in June Father’s Day. The idea was officially approved by President Woodrow Wilson in 1916. in 1924, President Calvin Coolidge recommended national observance of the occasion “to establish more intimate (亲密) relations between fathers and their children, and to impress upon fathers the full measure of their obligations.” The red or white rose is recognized as the official Father’s Day flower.Father’s Day took longer to establish on a national scale than Mother’s Day, but as the idea grained popularity, tradesmen and manufacturers began to see the commercial possibilities. They encouraged sons and daughters to honor their fathers with small thank-you presents, such as a tie or pair of socks, as well as by sending greeting cards.During the Second World War, American servicemen stationed in Britain began to request Father’s Day greeting cards to send home. This generated a response with British card publishers. Though at first the British public was slow to accept this rather artificial day, it’s now well celebrated in Britain on the third Sunday in June in much the same way as in America.Father’s Day seems to be much less important as occasion than the Mother’s Day. Not many of the children offer their fathers some presents. But the American fathers still think they are much better fatedthan the fathers of many other countries, who have not even a day for their sake in name only.11. When did Father’s Day officially begin to have national popularity?A. 1907B. 1909C. 1916D. 192412. Who first started the idea of holding the Father’s Day?A. Mrs. John Bruce DoddB. Mrs. John Bruce’s MotherC. The government of Washington.D. Some businessmen.13. What flower will be popular on Father’s Day?A. LilyB. Water LilyC. Red rose or white roseD. Sunflower.14. Which statement is true, a according to this passage?A. It took even longer for Mother’s Day to gain national popularity.B. The businessmen helped to make Father’s Day popular.C. Father’s Day is only celebrated in America.D. Father’s Day is only a trick of the businessmen to make money.15. What was the first reaction of the British publishing towards Father’s Day?A. They thought highly of it and accepted it at once.B. They just accepted it at once without any hesitation.C. They just thought it a joke.D. They thought it was too artificial and took a long time to accept.Passage 4(非英语类学生必做)Questions 16 to 20 are based on the following passage:Culture shock is an occupational disease (职业病) for people who have been suddenly transplanted abroad.Culture shock is caused by the anxiety that results from losing all familiar signs and symbols of social intercourse. Those signs are as following: when to shake hands and what to say when meet people, when and how to give tips, how to make purchases, when to accept and refuse invitations, when to take statements seriously and when not. These signs, which may be words, gestures, facial expressions, or customs, are acquired by all of us in the course of growing up and as much a part of our culture as the language we speak or the beliefs we accept. All of us depend on hundreds of these signs for our peace of mind and day-to-day efficiency, but we do not carry most at the level of conscious awareness.Now when an individual enters a strange culture, all or most of these familiar signs are removed. No matter how broadminded or full of good will you may be a series of supports have been knocked from under you, followed by a feeling of frustration. When suffering from culture shock people first reject the environment which caused discomfort. The ways of the host country are bad because they make us feel bad. When foreigners in a strange land get together in complain about the host country its people, you can be sure that they are suffering from culture shock.16. According to the passage, culture shock is .A. an occupational disease of foreign peopleB. may lead to very serious symptomsC. actually not a diseaseD. incurable17. According to the passage, culture shock result from .A. the sudden change of social atmosphere and customsB. the sudden change of our daily habitsC. the sudden loss of our own signs and symbolsD. the discomfort that we feel when faced with a foreigner18. Which one of the following may not be a symptom of culture shock?A. You don’t know how to express your gratitude.B. You d on’t know how to greet other people.C. You suddenly forget what a word means.D. You don’t understand why a foreigner shrugs.19. According to the passage, how would a person who stays abroad most probably react when he is frustrated by the culture shock?A. He is most likely to refuse to absorb the strange environment at first.B. He is really to accept the change and adapt himself to the new environment.C. Although he takes the culture difference for granted, he still doesn’t know how to do with it.D. He may begin to hate the people or things around him.20. The main idea of this passage is that .A. culture shock is an occupational diseaseB. culture shock is caused by the anxiety of living in a strange cultureC. culture shock has peculiar symptomsD. it is very hard to cope with life in a new setting(英语类学生必做)Questions 16 to 20 are based on the following passage:In a family where the roles of men and women are not sharply separated and where many household tasks are shared to a greater or lesser extent, notions of male superiority are hard to maintain. The pattern of sharing in tasks and in decisions makes for equality and this in turn leads to further sharing. In such a home, the growing boy and girl learn to accept equality more easily than did their parents and to prepare more fully for participation in a world characterized by cooperation rather by the “battle of the sexes”.If the process goes too far and man’s role is regarded as less important—and that has happened in some cases—we are as badly off as before, only in reverse.It is time to reassess the role of the man in the American family. We are getting a little tired of “Monism”—but we don’t want to exchange it for a “neo-Popism”. What we need, rather, is the recognition that bringing up children involves a partnership of equals. There are sings that psychiatrists, psychologists, social workers, and specialists on the family are becoming more aware of the part men play and that they have decided that women should not receive all the credit—nor the blame. We have almost given up saying that a woman’s place is in the home. We are beginning, however, to analyze man’s place in the home and to insist that he does have a place on it. Nor is that place irrelevant to the healthy development of the child.The family is a co-operative enterprise for which it is difficult to lay down rules, because each family needs to work out its own ways for solving its own problems.Excessive authoritarianism (命令主义) has unhappy consequences, whether it wears skirts or trousers, and the ideal of equal rights and equal responsibilities is pertinent (相关的，切题的) not only to a healthy democracy, but also to a healthy family.16. The ideal of equal rights and equal responsibilities is .A. fundamental to a sound democracyB. not pertinent to healthy family lifeC. responsible for MonismD. what we have almost given up17. The danger in the sharing of household tasks by the mother and the father is that .A. the role of the father may become an inferior oneB. the role of the mother may become an inferior oneC. the children will grow up believe that life is a battle of sexesD. sharing leads to constant arguing18. The author states that bringing up children .A. is mainly the mother’s jobB. belongs among the duties of the fatherC. is the job of schools and churchesD. involves a partnership of equals19. According to the author, the father’s role in the home is .A. minor because he is an ineffectual parentB. irrelevant to the healthy development of the childC. pertinent to the healthy development of the childD. identical to the role of the child’s mother20. With which of the following statements would the author be most likely to agree?A. A healthy, co-operative family is a basic ingredient of a healthy society.B. Men are basically opposed to sharing household chores.C. Division of household responsibilities is workable only in theory.D. A woman’s place is always in the home.Part II Vocabulary and Structure （共40小题，每小题1分，共40分）Directions: In this part there are forty incomplete sentences. Each sentence is followed by four choices. Choose the one that best completes the sentence and then mark your answer on the Answer Sheet.21. The teacher the students on a tour through the art museum.A. madeB. indicatedC. forcedD. took22. Tom’s parents died when he was a child, so he was by his relatives.A. grown upB. brought upC. raisedD. fed up23. Here is my card. Let’s keep in .A. touchB. relationC. connectionD. friendship24. So far there is no proof people from other planets do exist.A. whichB. howC. whatD. that25. The newspapers reported yesterday several on the boundaries of these two countries.A. incidentsB. happeningsC. eventsD. accidents26. We’ve worked out the plan and now we must put it into .A. factB. realityC. practiceD. deed27. He didn’t and so he failed the examination.A. work enough hardB. hard work enoughC. hard enough workD. work hard enough28. Not until Mr. Smith came to China what kind of country she is.A. he knewB. he didn’t knowC. did he knowD. he couldn’t know29. Scientists say it may be ten years this medicine was put to use.A. sinceB. beforeC. afterD. when30. In some countries, is called “equality” does not really mean equal rights for all people.A. thatB. whatC. whichD. how31. We didn’t know his telephone number, otherwise we him.A. would telephoneB. would have telephoneC. had telephonedD. must have telephoned32. We’ve missed the last bus, I’m afraid we have no but to take a taxi.A. wayB. possibilityC. choiceD. selection33. Luckily, most sheep the flood last month.A. enduredB. survivedC. livedD. passed34. My parents always let me have my own of living.A. wayB. methodC. mannerD. fashion35. Like other language skills, reading requires practice.A. the most ofB. much of theC. most of theD. more of the36. It is only through practice one will be able to swim skillfully.A. whatB. whoC. thatD. which37. The brain is capable of ignoring pain message of to concentrate on other activities.A. it allowedB. is it allowedC. allowedD. allowed it38. Don’t worry, I have already them the decision.A. informed; withB. informed; ofC. informed; forD. informed; that39. The child was sorry his mother when he arrived at the station.A. to missB. having missedC. missingD. to have missed40. I wonder why he to discuss the problem at the meeting.A. declinedB. rejectedC. refusedD. delayed41. You can hang up what you like on these walls.A. bareB. emptyC. blankD. vacant42. According to a , the majority would rather have newspapers without a government than a government without newspapers.A. electionB. campaignC. pollD. vote43. The population of the village has decreased 150 to 500.A. inB. atC. byD. with44. It seems that there is that I can’t do.A. nothingB. anythingC. everythingD. none45. They are often caring more about animals than human beings.A. accused ifB. accused withC. charged ofD. charged for46. a good beginning is made, the word is half done.A. As soon asB. WhileC. AsD. Once47. George could not his foolish mistake.A. account inB. count onC. count forD. account for48. We came into this field late, so we must work hard to the lost time.A. make up forB. make outC. keep up withD. put up with49. The new law will came into on the day it is passed.A. effectB. useC. serviceD. existence50. We can separate the mixture into the pure chemical compounds it is composed.A. in whichB. of whatC. of whichD. from which51. Mrs. Lincoln has that she is unable to get a job.A. such small educationB. so little educationC. a such little educationD. a so small education52. She can’t prevent her little boy shooting birds.A. from; toB. on; atC. with; upD. from; at53. Many countries are increasing their use of natural gas, wind and other forms of .A. energyB. sourceC. powerD. material54. A darkened sky in the daytime is usually and indication that a storm is .A. possible comingB. about to take placeC. close byD. expected to be severe55. We all know that speak louder than words.A. movementsB. performanceC. operationsD. actions56. , he could not cover the whole distance in fifteen minutes.A. Fast as he canB. As he can ran fastC. If he can ran fastD. Since he ran fast57. Agricultural production in that country has increased in recent years.A. vastlyB. strikinglyC. considerablyD. extremely58. Peter has planned to some money every month so that he can buy a used car next year.A. set asideB. set upC. set inD. set along59. Although I spoke to him many times, he never took any of what I said.A. attentionB. noticeC. warningD. observation60. They overcame all the difficulties and fulfilled the plan three months ahead of time, is something we had not expected.A. thatB. whatC. itD. whichPart III Cloze （共20小题，每小题1分，共20分）Directions: There are twenty blanks in the following passage. For each blank there are four choices. Choose the one that best fits into the passage and then marks your answer on the Answer Sheet.(非英语类学生必做)Most Americans don’t like to get advice from members of their family. When they need advice, they don’t usually 61 people they know. 62 , many Americans write letters to newspapers and magazines which give advice 63 many different subjects, including family problem, sex, the use 64 the language, health, cooking, children, and how to buy a house or a car.65 newspaper regularly print letters 66 readers with problems. Along 67 the letters there are answers written 68 people who are supposed to know how to 69 such problems. Some of these writers are doctors: 70 are lawyers or educators. But two of the most famous writers of advice 71 women without special training 72 this kind of work. One of them answers letters 73 to “Dear Abby”. The other is addressed 74 “Dear Ann Landers”. Experience is their preparation for 75 advice.There is one writer who has not lived long 76 to have much experience. She is a girl named Angel Cavaliere, who started writing 77 for newspaper readers 78 the age of ten, her advice to young readers now 79 regularly in the Philadelphia Bulletin in a column 80 DEAR ANGEL.61. A. talk B. ask C. tell D. speak62. A. Because B. Instead C. When D. As63. A. for B. in C. on D. with64. A. with B. on C. to D. of65. A. Most B. These C. Those D. The66. A. from B. for C. to D. about67. A. in B. with C. on D. for68. A. to B. for C. about D. by69. A. make B. overcome C. beat D. solve70. A. some B. many C. others D. those71. A. is B. are C. were D. was72. A. for B. on C. at D. by73. A. made B. addressed C. written D. sent74. A. with B. for C. as D. by75. A. producing B. giving C. making D. sending76. A. time B. yet C. way D. enough77. A. advise B. answers C. advice D. problems78. A. at B. on C. in D. about79. A. gives B. sends C. appears D. writes(英语类学生必做)There are two factors which determine an individual’s intelligence. The first is the sort of brain he is born 61 . Human brains differ considerably, 62 being more capable than others. 63 no matter how good a brain he has to begin with, an individual will have a low order of intelligence 64 he has opportunities to learn. So the second factor is what 65 to the individual—the sort of environment in which he is brought 66 . If an individual is handicapped (受阻碍) 67 , it is likely that his brain will 68 to develop and he will 69 attain the level of intelligence of which he is 70 .The importance of environment in determining an individual’s intelligence can be 71 by the case history of the identical twins, Peter and John. When the twins were three months old, their parents died, and they are placed in 72 foster (寄养) homes. Peter was reared by parents of low intelligence in an 73 community with poor educational 74 . John, 75 , was educated in the home of well-to-do parents who has been to college. This environmental 76 continued until the twins were 77 their late teens, 78 they were given tests to 79 their intelligence. John’s I.Q. (智商) was 125, twenty-five points higher than the 80 andfully forty points higher than his identical brother.61. A. for B. by C. with D. in62. A. most B. some C. many D. few63. A. But B. For C. Still D. And64. A. if B. thought C. as D. unless65. A. refers B. applies C. happens D. concerns66. A. about B. up C. forward D. forth67. A. relatively B. intelligently C. regularly D. environmentally68. A. fail B. help C. manage D. stop69. A. ever B. never C. even D. nearly70. A. able B. capable C. available D. acceptable71. A. demonstrated B. denied C. neglected D. ignored72. A. separate B. similar C. remote D. individual73. A. omitted B. isolated C. enclosed D. occupied74. A. possibilities B. opportunities C. capacities D. responsibilities75. A. moreover B. consequently C. then D. however76. A. exception B. division C. difference D. alteration77. A. in B. by C. at C. for78. A. while B. since C. when D. because79. A. estimate B. count C. decide D. measure80. A. average B. common C. usual D. ordinaryPart IV Translation （共35分）Section A （共5小题，每小题4分，共20分）81、The captain realized that the men tried to deceive him so he made them work very hard for the rest of the voyage. (Passage One)82、By making vehicles lighter in weight aluminum has greatly reduced the amount of fuel needed to move them, (Passage Two)83、As the source of aluminum is almost inexhaustible, we can expect that more and more uses will be found for this versatile metal. (Passage Two)84、（非英语类学生必做）Not many of the children offer their fathers some presents. But the American fathers still think they are much better fated than the fathers of many other countries, who have not even a day for their sake in name only. (Passage Three)85、（非英语类学生必做）Culture shock is caused by the anxiety that results from losing all familiar signs and symbols of social intercourse. (Passage Four)84、（英语类学生必做）In a family where the roles of men and women are not sharply separated and where many household tasks are shared to a greater or lesser extent, notions of male superiority are hard to maintain. (Passage Four) 85、（英语类学生必做）Excessive authoritarianism (命令主义) has unhappy consequences, whether it wears skirts or trousers, and the ideal of equal rights and equal responsibilities is pertinent (相关的，切题的) not only to a healthy democracy, but also to a healthy family. (Passage Four)Section B （共5小题，每小题3分，共15分）Directions: Translate the following sentences into English.86、如果你听从我的劝告，你可能会获胜。

2004高考数学试题(江苏)及答案

)2004年普通高等学校招生江苏卷数学试题一、选择题(5分×12=60分)1.设集合P={1，2，3，4}，Q={R x x x ∈≤,2}，则P ∩Q 等于 ( )(A){1，2} (B) {3，4} (C) {1} (D) {-2，-1，0，1，2}2.函数y=2cos 2x+1(x ∈R )的最小正周期为 ( )(A)2π(B)π (C)π2 (D)π43.从4名男生和3名女生中选出4人参加某个座谈会，若这4人中必须既有男生又有女生，则不同的选法共有 ( )(A)140种 (B)120种 (C)35种 (D)34种4.一平面截一球得到直径是6cm 的圆面，球心到这个平面的距离是4cm ，则该球的体积是 ( )(A)33π100cm (B) 33π208cm (C) 33π500cm (D) 33π3416cm5.若双曲线18222=-by x 的一条准线与抛物线x y 82=的准线重合，则双曲线离心率为 ( ) (A)2 (B)22 (C) 4 (D)246.某校为了了解学生的课外阅读情况，随机调查了50名学生，得到他们在某一天各自课外阅读所用时间的数据，结果用右侧的条形图表示. 根据条形图可得这50名学生这一天平均每人的课外阅读时间为 ( ) (A)0.6小时 (B)0.9小时 (C)1.0小时 (D)1.5小时7.4)2(x x +的展开式中x 3的系数是 ( )(A)6 (B)12 (C)24 (D)488.若函数)1,0)((log ≠>+=a a b x y a 的图象过两点(-1，0)和(0，1)，则(A)a=2,b=2 (B)a= 2 ,b=2 (C)a=2,b=1 (D)a= 2 9.将一颗质地均匀的骰子（它是一种各面上分别标有点数1，2，3，4，3次，至少出现一次6点向上和概率是 ( )(A)5216 (B)25216 (C)31216 (D)9121610.函数13)(3+-=x x x f 在闭区间[-3，0]上的最大值、最小值分别是 ( ) (A)1，-1 (B)1，-17 (C)3，-17 (D)9，-1911.设k>1，f(x)=k(x-1)(x ∈R ) . 在平面直角坐标系xOy 中，函数y=f(x)的图象与x 轴交于A 点，它的反函数y=f -1(x)的图象与y 轴交于B 点，并且这两个函数的图象交于P 点. 已知四边形OAPB 的面积是3，则k 等于 ( )(A)3 (B)32 (C)43 (D)6512.设函数)(1)(R x xxx f ∈+-=，区间M=[a ，b](a<b)，集合N={M x x f y y ∈=),(}，则使M=N 成立的实数对(a ，b)有 ( )(A)0个 (B)1个 (C)2个 (D)无数多个二、填空题(4分×4=16分)13.二次函数y=ax 2+bx+c(x ∈R )的部分对应值如右表：则不等式ax 2+bx+c>0的解集是_____________________.14.以点(1，2)为圆心，与直线4x+3y-35=0相切的圆的方程是________________.15.设数列{a n }的前n 项和为S n ，S n =)13(1-n a (对于所有n ≥1)，且a 4=54，则a 1的数值是_______________________.16.平面向量b a ,中，已知=(4，-3)=1，且⋅=5，则向量=__________. 三、解答题(12分×5+14分=74分)17.已知0<α<2π，tan 2α+cot 2α=25，求sin(3πα-)的值.18.在棱长为4的正方体ABCD-A 1B 1C 1D 1中，O 是正方形A 1B 1C 1D 1的中心，点P 在棱CC 1上，且CC 1=4CP.(Ⅰ)求直线AP 与平面BCC 1B 1所成的角的大小（结果用反三角函数值表示）； (Ⅱ)设O 点在平面D 1AP 上的射影是H ，求证：D 1H ⊥AP ； (Ⅲ)求点P 到平面ABD 1的距离.19.制定投资计划时，不仅要考虑可能获得的盈利，而且要考虑可能出现的亏损.某投资人打算投资甲、乙两个项目.根据预测，甲、乙项目可能的最大盈利率分别为100﹪和50﹪，可能的最大亏损分别为30﹪和10﹪. 投资人计划投资金额不超过10万元，要求确保可能的资金亏损不超过1.8万元. 问投资人对甲、乙两个项目各投资多少万元，才能使可能的盈利最大？ 20.设无穷等差数列{a n }的前n 项和为S n .(Ⅰ)若首项=1a 32 ，公差1=d ，求满足2)(2k kS S =的正整数k ；(Ⅱ)求所有的无穷等差数列{a n }，使得对于一切正整数k 都有2)(2k kS S =成立.21.已知椭圆的中心在原点，离心率为12 ，一个焦点是F （-m,0）(m 是大于0的常数). (Ⅰ)求椭圆的方程；(Ⅱ)设Q 是椭圆上的一点，且过点F 、Q 的直线l 与y 轴交于点M. =，求直线l 的斜率. 22.已知函数))((R x x f ∈满足下列条件：对任意的实数x 1，x 2都有)]()()[()(λ2121221x f x f x x x x --≤-和2121)()(x x x f x f -≤-，其中λ是大于0的常数.设实数a 0，a ，b 满足0)(0=a f 和)(λa f a b -=(Ⅰ)证明1λ≤，并且不存在00a b ≠，使得0)(0=b f ； (Ⅱ)证明20220))(λ1()(a a a b --≤-； (Ⅲ)证明222)]()[λ1()]([a f b f -≤.· B 1P A C D A 1 C 1D 1 BO H·2004年普通高等学校招生江苏卷数学试题参考答案一、选择题：ABDCA BCADC BA 二、填空题；13、{2x x <-或3}x >； 14、22(1)(1)25x y -+-=； 15、2； 16、43(,)55b =-。

2004年成人高考专升本高等数学二考试真题附参考答案

2004年成人高考专升本高等数学二考试真题及参考答案
一、选择题：本大题共5个小题，每题4分，共20分，在每题给出的四个选项中，只有一项为哪一项符合题目的要求，把所选项前的字母填在题后的括号内。

第1题
参考答案：A
第2题
参考答案：D
第3题
参考答案：D
第4题
参考答案：B
第5题
参考答案：C
二、填空题：本大题共10个小题，每题4分，共40分，把答案填写在题中横线上。

第6题
参考答案：1
第7题
参考答案：0
第8题
参考答案：1
第9题
参考答案：2/x3
第10题
参考答案：-1
第11题
参考答案：0
第12题
参考答案：e-1 第13题
参考答案：1
第14题
参考答案：-sinx 第15题
三、解答题：本大题共13个小题，共90分，解容许写出推理、演算步骤. 第16题
第17题
第18题
第19题
第20题
第21题
第22题
第23题
第24题
第25题
第26题
第27题
第28题。

2001—2004年江苏专转本高数真题(打印版)

2001年江苏省普通高校“专转本”统一考试高等数学一、选择题（本大题共5小题，每小题3分，共15分；在每小题给出的四个选项中，只有一项是符合要求的，请把所选项前的字母填在题后的括号内）1、下列各极限正确的是A 、e x x x =+→)11(lim 0B 、e xxx =-→)11(lim 0C 、11sin lim =∞→x x xD 、11sin lim 0=→xx x2、不定积分=-⎰dx x 211 A 、211x- B 、c x+-211 C 、x arcsin D 、c x +arcsin3、若)()(x f x f -=，在[)+∞,0内0)('>x f 、0)(''>x f ，则在)0,(-∞内必有A 、0)('<x f ,0)(''<x f B 、0)('<x f ,0)(''>x f C 、0)('>x f ,0)(''<x f D 、0)('>x f ,0)(''>x f4、=-⎰dx x 21A 、0B 、2C 、－1D 、15、方程x y x 422=+在空间直角坐标系中表示A 、圆柱面B 、点C 、圆D 、旋转抛物面二、填空题（本大题共5小题，每小题3分，共15分，）6、设⎩⎨⎧+==22t t y te x t ，则==0t dx dy7、0136'''=+-y y y 的通解为 8、交换积分次序=⎰⎰dy y x f dx xx220),(9、函数yx z =的全微分=dz10、设)(x f 为连续函数，则=+-+⎰-dx x x x f x f 311])()([三、计算题（本大题共10小题，每小题4分，共40分） 11、已知5cos)21ln(arctanπ+++=x x y ，求dy .12、计算xx dte x xt x sin lim22⎰-→.13、求)1(sin )1()(2--=x x xx x f 的间断点，并说明其类型.14、已知xyx y ln 2+=，求11==y x dx dy . 15、计算dx e e xx⎰+12. 16、已知⎰∞-=+02211dx x k ，求k 的值.17、求x x y y sec tan '=-满足00==x y 的特解.18、计算⎰⎰Ddxdy y 2sin ，D 是1=x 、2=y 、1-=x y 围成的区域.19、已知)(x f y =过坐标原点，并且在原点处的切线平行于直线032=-+y x ，若b ax x f +=2'3)(，且)(x f 在1=x 处取得极值，试确定a 、b 的值，并求出)(x f y =的表达式.20、设),(2y x x f z =，其中f 具有二阶连续偏导数，求x z ∂∂、yx z ∂∂∂2. 四、综合题（本大题共4小题，第21小题10分，第22小题8分，第23、24小题各6分，共30分）21、过)0,1(P 作抛物线2-=x y 的切线，求（1）切线方程；（2）由2-=x y ，切线及x 轴围成的平面图形面积；（3）该平面图形分别绕x 轴、y 轴旋转一周的体积。

2004年江苏专转本高等数学真题(附答案)

2004年江苏省普通高校“专转本”统一考试高等数学一、单项选择题（本大题共6小题，每小题3分，满分18分.）1、[](]⎩⎨⎧∈--∈=2,00,3)(33x xx x x f ，是：（）A 、有界函数B 、奇函数C 、偶函数D 、周期函数2、当0→x 时，x x sin 2-是关于x 的（） A 、高阶无穷小B 、同阶但不是等价无穷小C 、低阶无穷小D 、等价无穷小3、直线L 与x 轴平行且与曲线xe x y -=相切，则切点的坐标是（） A 、()1,1B 、()1,1-C 、()1,0-D 、()1,04、2228R y x =+设所围的面积为S ，则dx x R R⎰-220228的值为（）A 、SB 、4S C 、2S D 、S 25、设yx y x u arctan),(=、22ln ),(y x y x v +=，则下列等式成立的是（） A 、y v x u ∂∂=∂∂ B 、xv x u ∂∂=∂∂ C 、xv y u ∂∂=∂∂ D 、yv y u ∂∂=∂∂ 6、微分方程xxe y y y 22'3''=+-的特解*y 的形式应为（）A 、xAxe 2B 、xe B Ax 2)(+ C 、xeAx 22 D 、x e B Ax x 2)(+二、填空题（本大题共6小题，每小题3分，满分18分）7、设xx x x f ⎪⎭⎫⎝⎛++=32)(，则=∞→)(lim x f x 8、过点)2,0,1(-M 且垂直于平面2324=-+z y x 的直线方程为9、设)()2)(1()(n x x x x x f +++= ，N n ∈，则=)0('f 10、求不定积分=-⎰dx xx 231arcsin11、交换二次积分的次序=⎰⎰-dy y x f dx x x 212),(12、幂级数∑∞=-12)1(n nnx 的收敛区间为三、解答题（本大题共8小题，每小题5分，满分40分） 13、求函数xxx f sin )(=的间断点，并判断其类型. 14、求极限)31ln()1()sin (tan lim22x edtt t x xx +--⎰→.15、设函数)(x y y =由方程1=-yxe y 所确定，求22=x dx yd 的值.16、设)(x f 的一个原函数为xe x，计算⎰dx x xf )2('.17、计算广义积分dx x x ⎰+∞-211.18、设),(xy y x f z -=，且具有二阶连续的偏导数，求x z ∂∂、yx z∂∂∂2.19、计算二重积分dxdy y yD⎰⎰sin ，其中D 由曲线x y =及x y =2所围成. 20、把函数21)(+=x x f 展开为2-x 的幂级数，并写出它的收敛区间. 四、综合题（本大题共3小题，每小题8分，满分24分） 21、证明：⎰⎰=πππ)(sin 2)(sin dx x f dx x xf ，并利用此式求dx xxx⎰+π2cos 1sin .22、设函数)(x f 可导，且满足方程)(1)(2x f xdt t tf x++=⎰，求)(x f .23、甲、乙二城位于一直线形河流的同一侧，甲城位于岸边，乙城离河岸40公里，乙城在河岸的垂足与甲城相距50公里，两城计划在河岸上合建一个污水处理厂，已知从污水处理厂到甲乙二城铺设排污管道的费用分别为每公里500、700元。

江苏专升本高等数学真题(附答案)

江苏专转本高数考纲及重点总结一、函数、极限和连续（一）函数（1）理解函数的概念：函数的定义，函数的表示法，分段函数。

（2）理解和把握函数的简单性质：单调性，奇偶性，有界性，周期性。

（3）了解反函数：反函数的定义，反函数的图象。

（4）把握函数的四则运算与复合运算。

（5）理解和把握基本初等函数：幂函数，指数函数，对数函数，三角函数，反三角函数。

（6）了解初等函数的概念。

重点：函数的单调性、周期性、奇偶性，分段函数和隐函数（二）极限（1）理解数列极限的概念：数列，数列极限的定义，能根据极限概念分析函数的变化趋势。

会求函数在一点处的左极限与右极限，了解函数在一点处极限存在的充分必要条件。

（2）了解数列极限的性质：唯一性，有界性，四则运算定理，夹逼定理，单调有界数列，极限存在定理，把握极限的四则运算法则。

（3）理解函数极限的概念：函数在一点处极限的定义，左、右极限及其与极限的关系，x趋于无穷（x→∞，x→+∞，x→-∞）时函数的极限。

（4）把握函数极限的定理：唯一性定理，夹逼定理，四则运算定理。

（5）理解无穷小量和无穷大量：无穷小量与无穷大量的定义，无穷小量与无穷大量的关系，无穷小量与无穷大量的性质，两个无穷小量阶的比较。

（6）熟练把握用两个重要极限求极限的方法。

重点：会用左、右极限求解分段函数的极限，把握极限的四则运算法则、利用两个重要极限求极限以及利用等价无穷小求解极限。

（三）连续（1）理解函数连续的概念：函数在一点连续的定义，左连续和右连续，函数在一点连续的充分必要条件，函数的中断点及其分类。

（2）把握函数在一点处连续的性质：连续函数的四则运算，复合函数的连续性，反函数的连续性，会求函数的中断点及确定其类型。

（3）把握闭区间上连续函数的性质：有界性定理，最大值和最小值定理，介值定理（包括零点定理），会运用介值定理推证一些简单命题。

（4）理解初等函数在其定义区间上连续，并会利用连续性求极限。

重点：理解函数（左、右连续）性的概念，会判别函数的中断点。

2004年高考江苏卷数学试题及答案

122 普通高等学校招生全国统一考试数学（江苏卷）一、选择题(5 分×12=60 分)1.设集合 P={1，2，3，4}，Q={ x x ≤ 2, x ∈ R }，则 P ∩Q 等于()(A){1，2} (B) {3，4} (C) {1} (D) {-2，-1，0，1，2} 2.函数 y=2cos 2x+1(x ∈R )的最小正周期为( )(A)π (B) π (C) 2π 2(D) 4π3.从 4 名男生和 3 名女生中选出 4 人参加某个座谈会，若这 4 人中必须既有男生又有女生，则不同的选法共有 ( ) (A)140 种 (B)120 种 (C)35 种 (D)34 种4.一平面截一球得到直径是 6cm 的圆面，球心到这个平面的距离是 4cm ，则该球的体积是( ) (A)100π cm 33x2(B)y 2208π cm 33(C)500π cm 332(D)416 33π cm 35. 若双曲线-8 b 2= 1 的一条准线与抛物线 y = 8x 的准线重合，则双曲线离心率为()(A) (B) 2 (C) 4 (D)46.某校为了了解学生的课外阅读情况，随机调查了 50 名学生，得到他们在某一天各自课外阅读所用时间的数据，结果用右侧的条形图表示. 根据条形图可得这 50 名学生这一天平均每人的课外阅读时间为 ( ) (A)0.6 小时 (B)0.9 小时 (C)1.0 小时 (D)1.5 小时时间(小时)7. (2x + x ) 4的展开式中 x 3 的系数是( )221 +x(A)6 (B)12 (C)24 (D)48 8.若函数 y = log a (x + b )(a > 0, a ≠ 1) 的图象过两点(-1，0)和(0，1)，则( )(A)a=2,b=2 (B)a= 2 ,b=2 (C)a=2,b=1 (D)a= 2 ,b= 29.将一颗质地均匀的骰子（它是一种各面上分别标有点数 1，2，3，4，5，6 的正方体玩具）先后抛掷 3 次，至少出现一次 6 点向上和概率是 ( ) (A) 5 216 (B) 25 216 (C) 31 216 (D) 91 21610.函数 f (x ) = x 3 - 3x +1 在闭区间[-3，0]上的最大值、最小值分别是 ( )(A)1，-1 (B)1，-17 (C)3，-17 (D)9，-19 11.设 k>1，f(x)=k(x-1)(x ∈R ) . 在平面直角坐标系 xOy 中，函数 y=f(x)的图象与 x 轴交于 A点，它的反函数 y=f -1(x)的图象与 y 轴交于 B 点，并且这两个函数的图象交于 P 点. 已知四边形 OAPB 的面积是 3，则 k 等于 ( )(A)3 (B)3 2 (C)4 3 (D)65 12.设函数 f (x ) = -x(x ∈ R ) ，区间 M=[a ，b](a<b)，集合 N={ y y = f (x ), x ∈ M }，则使M=N 成立的实数对(a ，b)有 ()(A)0 个 (B)1 个 (C)2 个(D)无数多个二、填空题(4 分×4=16 分)13.二次函数 y=ax 2+bx+c(x ∈R )的部分对应值如下表：则不等式 ax 2+bx+c>0 的解集是 . 14.以点(1，2)为圆心，与直线 4x+3y-35=0 相切的圆的方程是.a 1 (3n -1)15.设数列{a n }的前 n 项和为 S n ，S n =2.(对于所有 n ≥1)，且 a 4=54，则 a 1 的数值是16.平面向量 a , b 中，已知 a =(4，-3)，，且 a ⋅ b =5，则向量b = .三、解答题(12 分×5+14 分=74 分)17.已知 0<α< π，tan α+cot α = 5，求 sin( α - π)的值.222 2318.在棱长为 4 的正方体 ABCD-A 1B 1C 1D 1 中，O 是正方形 A 1B 1C 1D 1 的中心，点 P 在棱 CC 1上，且CC1=4CP.34k k3 (Ⅰ)求直线 AP 与平面 BCC 1B 1 所成的角的大小（结果用反三角函数值表示）； (Ⅱ)设 O 点在平面 D 1AP 上的射影是 H ，求证：D 1H ⊥AP ； D 1 C 1(Ⅲ)求点 P 到平面 ABD 1 的距离.·OA 1B 119.制定投资计划时，不仅要考虑可能获得的盈利，而且要考虑可D P损. C某投资人打算投资甲、乙两个项目. 根据预测，甲、乙项目可能的最大盈利率分别为 100 ﹪和 50﹪，可能的最大亏损分别为 30﹪和 10﹪. 投资人计AB求确保可能的资金亏损不超过 1.8 万元. 问投资人对甲、乙两个项目各投资多少万元，才能使可能的盈利最大？20.设无穷等差数列{a n }的前 n 项和为 S n .(Ⅰ)若首项 a 1 = 2，公差 d = 1 ，求满足 S 2 = (S k ) 2的正整数 k ； (Ⅱ)求所有的无穷等差数列{a n }，使得对于一切正整数 k 都有 S 2 = (S k ) 2 成立.21.已知椭圆的中心在原点，离心率为1 2，一个焦点是 F （-m,0）(m 是大于 0 的常数).(Ⅰ)求椭圆的方程；(Ⅱ)设 Q 是椭圆上的一点，且过点 F 、Q 的直线l 与 y 轴交于点 M. 若= 2 QF ，求直线l 的斜率.22.已知函数 f (x )(x ∈ R ) 满足下列条件：对任意的实数 x 1，x 2 都有·H MQ 能出现的亏5)⎨λ(x 1 - x 2 ) 2 ≤ (x 1 - x 2 )[ f (x 1 ) - f (x 2 )] 和 f (x 1 ) - f (x 2 ) ≤ x 1 - x 2，其中 λ 是大于 0 的常数.设实数 a 0，a ，b 满足 f (a 0 ) = 0 和b = a - λf (a )(Ⅰ)证明 λ ≤ 1 ，并且不存在b 0 ≠ a 0 ，使得 f (b 0 ) = 0 ； (Ⅱ)证明(b - a 0 ) 2 ≤ (1 - λ2 )(a - a 0 ) 2 ；(Ⅲ)证明[ f (b )]2 ≤ (1 - λ2 )[ f (a )]2 .一、选择题2004 年普通高等学校招生全国统一考试数学（江苏卷）参考答案ABDCA BCADC BA 二、填空题13、{x x < -2 或 x > 3}14、(x -1)2+ ( y -1)2= 25 15、24 3 16、b = ( , - )5 5三、解答题17、解：由题意可知sin α= 4，5∴sin(α- π = 3 1018、解（1） ∠APB = arctan 41717（2）略（3）32219、解： ⎧ x + y ≤ 10，设 z = x + 0.5y⎩3x + y ≤ 184 - 3 366 ⎨y = 6 + = 当 ⎧ x = 4 时， z 取最大值 7 万元 ⎩20、解：（1） k = 4⎧a 1 = 0 ⎧a 1 = 1 ⎧a 1 =1 （2） ⎨ d = 0 或⎨d = 2或 ⎨d = 0 ⎩⎩ ⎩21、解：（1） x 24m 2 y 2 3m2 1（2） k = ±2 或 022、解：（1）不妨设 x > x ，由λ(x - x )2≤ (x - x ) ⋅ [ f (x ) - f (x ) ]12121212可知 f (x 1 ) - f (x 2 ) > 0 ，∴ f (x ) 是 R 上的增函数∴不存在b 0 ≠ a 0 ，使得 f (b 0 ) = 0又 λ(x - x )2≤ (x - x ) ⋅ [ f (x ) - f (x ) ]≤ (x - x )212121212∴λ≤ 1（2）要证： (b - a )2 ≤ (1- λ2)(a - a )2即证：λ⎡⎣(a - a 0 )2+ f 2(a )⎤⎦ ≤ 2 f (a )(a - a 0 )不妨设 a > a 0 ，(*)由λ(x - x )2≤ (x - x ) ⋅ [ f (x ) - f (x ) ]121212得 f (a ) - f (a 0 ) ≥ λ(a - a 0 ) ，即 f (a ) ≥ λ(a - a 0 ) ，70 0 0 0则 2 f (a )(a - a ) ≥ 2λ(a - a ) 2（1）由 f (x 1 ) - f (x 2 ) ≤ x 1 - x 2 得 f (a ) - f (a 0 ) ≤ a - a 0即 f (a ) ≤ a - a 0 ，则λ⎡⎣(a - a 0 )2+ f 2(a )⎤⎦ ≤ 2λ(a - a 0 )2（2）由（1）（2）可得λ⎡⎣(a - a 0 )2+ f 2(a )⎤⎦ ≤ 2 f (a )(a - a 0 )∴(b - a )2 ≤ (1- λ2)(a - a )2（3） [ f (a )]2 ≤ (a - a )2,∴(1- λ2 )[ f (a )]2 ≤ (1- λ2 )(a - a )2[ f (b )]2 ≤ (b - a )2又由（2）中结论(b - a )2≤ (1- λ2)(a - a )2 0∴[ f (b )]2 ≤ (1- λ2 )[ f (a )]2。

2019—2019年江苏专转本高数真题（打印版）共18页

2019—2019年江苏专转本⾼数真题（打印版）共18页第 1 页2005年江苏省普通⾼校“专转本”统⼀考试⾼等数学⼀、选择题（本⼤题共6⼩题，每⼩题4分，满分24分.）1、0=x 是xx x f 1sin )(=的A 、可去间断点B 、跳跃间断点C 、第⼆类间断点D 、连续点2、若2=x 是函数)21ln(ax x y +-=的可导极值点，则常数=aA 、1-B 、21C 、21- D 、13、若?+=C x F dx x f )()(，则?=dx x xf )(cos sinA 、C x F +)(sinB 、C x F +-)(sin C 、C F +(cos)D 、C x F +-)(cos 4、设区域D 是xoy 平⾯上以点)1,1(A 、)1,1(-B 、)1,1(--C 为顶点的三⾓形区域，区域1D 是D 在第⼀象限的部分，则：=+??dxdy y x xy D)sin cos (A 、??1)sin (cos 2D dxdy y xB 、??12D xydxdyC 、??+1)sin cos (4D dxdy y x xy D 、05、设yxy x u arctan ),(=，22ln ),(y x y x v +=，则下列等式成⽴的是v x u ??=?? B 、xvx u ??=C 、x v y u ??=??D 、yv y u ??=??6、正项级数(1) ∑∞=1n n u 、(2) ∑∞=13n n u ，则下列说法正确的是A 、若（1）发散、则（2）必发散B 、若（2）收敛、则（1）必收敛C 、若（1）发散、则（2）不定D 、若（1）、（2）敛散性相同⼆、填空题（本⼤题共6⼩题，每⼩题4分，满分24分）第 2 页7、=----→x x xe e x x x sin 2lim； 8、函数x x f ln )(=在区间[]e ,1上满⾜拉格郎⽇中值定理的=ξ；9、=++?-11211x x π；10、设向量{}2,4,3-=α、{}k ,1,2=β；α、β互相垂直，则=k ；11、交换⼆次积分的次序=?-+-dy y x f dx x x 2111),( ；12、幂级数∑∞=-1)12(n n x n 的收敛区间为；13、设函数+=a xx x f x F sin 2)()( 00=≠x x 在R 内连续，并满⾜：0)0(=f 、6)0('=f ，求a .14、设函数)(x y y =由⽅程?-==t t t y t x cos sin cos 所确定，求dx dy、22dx y d .15、计算?xdx x sec tan 3.16、计算?10arctan xdx17、已知函数),(sin 2y x f z =，其中),(v u f 有⼆阶连续偏导数，求xz、y x z2 18、求过点)2,1,3(-A 且通过直线12354:zy x L =+=-的平⾯⽅程. 19、把函数222)(xx x x f --=展开为x 的幂级数，并写出它的收敛区间.20、求微分⽅程0'=-+x e y xy 满⾜e y x ==1的特解.四、证明题（本题8分）21、证明⽅程：0133=+-x x 在[]1,1-上有且仅有⼀根.第 3 页五、综合题（本⼤题共4⼩题，每⼩题10分，满分30分） 22、设函数)(x f y =的图形上有⼀拐点)4,2(P ，在拐点处的切线斜率为3-，⼜知该函数的⼆阶导数a x y +=6''，求)(x f .23、已知曲边三⾓形由x y 22=、0=x 、1=y 所围成，求：（1）、曲边三⾓形的⾯积；（2）、曲边三⾓形饶X 轴旋转⼀周的旋转体体积.24、设)(x f 为连续函数，且1)2(=f ，dx x f dy u F uyu=)()(1，)1(>u（1）、交换)(u F 的积分次序；（2）、求)2('F .⾼等数学参考答案1、A2、C3、D4、B5、A6、C7、2 8、1-e 9、2π10、5 11、dx y x f dy y y ??---11102),( 12、)1,1(-13、因为)(x F 在0=x 处连续，所以)0()(lim 0F x F x =→，8262)0(2)0()(sin 2)()('0lim limlim =+=+=+-=+=→→→f x f x f x x x f x F x x x a F =)0(，故8=a .14、t t t t t t dtdx dt dydx dy -=-+-==sin sin cos cos ，t t x y dx y d t t csc sin 1)('''22=--==. 15、原式C x x x x xd x d x +-=-=-=??sec sec 31sec sec sec sec )1(sec 322.16、原式??++-=+-=102210211)1(2141arctan x x d dx x x x x π 102)1ln(214x +-=π2ln 214-=π 17、'z ?=??，''12''122cos 2)2(cos xf y y f x y x z =?= 18、{}1,2,5=l ，{}0,3,4-=B ，{}2,4,1-= {}22,9,8241125--=-=?=kj il π平⾯点法式⽅程为：0)2(22)1(9)3(8=+----z y x ，即592298=--z y x .19、x x x x x x x x f -?++?=-++=1132116)1121(3)(222nn n n x x ∑∞=+??+-=01212)1(3，收敛域为11<<-x . 20、xe y x y x=?+1'，通解为第 5 页x e x C C dx e x e e y x dx x x dx x +=+=-11 因为e y =)1(，C e e +=，所以0=C ，故特解为xey x=.21、证明：令13)(3+-=x x x f ，[]1,1-∈x ，且03)1(>=-f ，01)1(<-=f ，0)1()1(由连续函数零点定理知，)(x f 在)1,1(-上⾄少有⼀实根. （提醒：本题亦可⽤反证法证明）22、设所求函数为)(x f y =，则有4)2(=f ，3)2('-=f ，0)2(''=f .因为126''-=x y ，故12'123C x x y +-=，由3)2('-=y ，解得91=C . 故22396C x x x y ++-=，由4)2(=y ，解得22=C . 所求函数为：29623++-=x x x y . 23、（1）61612113102===?y dy y S （2）4021)()21(2212πππ=-=-=?x x dx x V x24、解：积分区域D 为：u y ≤≤1，u x y ≤≤（1）-===uxuDdx x f x dy x f dx d x f u F 111)()1()()()(σ；（2）)()1()('u f u u F -=，1)2()2()12()2('==-=f f F .2006年江苏省普通⾼校“专转本”统⼀考试⾼等数学参考答案1、C2、B3、C4、C5、C6、A7、2 8、)(0x f 9、1- 10、1 11、)cos sin (x x y e xy + 12、113、原式3221==--→x xx 14、21211122''t t t t x y dx dy tt =++-==，t t t t x dx dy dx y d t 411221)(22''22+=+== 15、原式C x x d x ++=++=?23 )ln 1(32)ln 1(ln 1第 6 页16、原式x d x dx x x xx x d x cos 24sin 2sin sin 20220202202+=-==πππππ24cos 2cos 24220202-=-+=πππx17、⽅程变形为2'-=x y x y y ，令x y p =则''xp p y +=，代⼊得：2'p xp -=，分离变量得：dx x dp p ??=-112，故C x p +=ln 1，C x x y +=ln . 18、令)1ln()(x x g +=，0)0(=g ，200'1)1()1()(+∞=∞=∑∑+-=-=n n n n nn x n dx x x g ，故201)1()(+∞=∑+-=n n n x n x f ，11<<-x . 19、{}1,1,11-n 、{}1,3,42-n ，k j i kj i n n l ++=--=?=3213411321直线⽅程为123123+=-=-z y x . 20、'22f x yz=??， ''222''213'2''22''212'2222)2(2yf x f x xf y f x f x xf xy z ++=?+?+=. 21、令33)(x x x f -=，[]2,2-∈x ，033)(2'=-=x x f ，1±=x ，2)1(-=-f ，2)1(=f ，2)2(-=f ，2)2(=-f ；所以2min -=f ，2max =f ，故2)(2≤≤-x f ，即233≤-x x .22、y x y +=2'，0)0(=y通解为x Ce x y +--=)22(，由0)0(=y 得2=C ，故x e x y 222+--=. 23、（1）364)8(2222=--=?-dx x x S （2）πππ16)8()(284240=-+=??dy y dy y V 24、dx x f t dy x f dx dxdy x f tt t D t==000)()()(=≠=?00)()(0t a（1）0)(lim )(lim 00==?→→dx x f t g tt t ，由)(t g 的连续性可知0)(lim )0(0===→t g g a t （2）当0≠t 时，)()('t f t g =，第 7 页当0=t 时，)0()(lim )(lim )0()(lim )0(000'f h f hdx x f hg h g g h hh h ===-=→→→?综上，)()('t f t g =.2006年江苏省普通⾼校“专转本”统⼀考试⾼等数学⼀、选择题（本⼤题共6⼩题，每⼩题4分，满分24分.）1、若21)2(lim 0=→x x f x ，则=→)3(lim0x f x x A 、21 B 、2 C 、3 D 、312、函数=≠=001sin)(2x x xx x f 在0=x 处A 、连续但不可导B 、连续且可导C 、不连续也不可导D 、可导但不连续3、下列函数在[]1,1-上满⾜罗尔定理条件的是A 、x e y =C 、21x y -=D 、xy 11-= 4、已知C e dx x f x +=?2)(，则=-?dx x f )('A 、C e x +-22B 、C e x +-221C 、C e x +--22D 、C e x +--2215、设∑∞=1n n u 为正项级数，如下说法正确的是A 、如果0lim 0=→n n u ，则∑∞=1n n u 必收敛B 、如果l u u nn n =+∞→1lim)0(∞≤≤l ，则∑∞=1n n u 必收敛 C 、如果∑∞=1n n u ，则∑∞=12n nu 必定收敛 D 、如果∑∞=-1)1(n n nu ，则∑∞=1n n u 必定收敛=1D }0,0,1|),{(22≥≥≤+y x y x y x ，则??=Ddxdy y x f ),(A 、0B 、??1),(D dxdy y x f C 、2??1),(D dxdy y x f D 、4??1),(D dxdy y x f⼆、填空题（本⼤题共6⼩题，每⼩题4分，满分24分）第 8 页7、已知0→x 时，)cos 1(x a -与x x sin 是等级⽆穷⼩，则=a 8、若A x f x x =→)(lim 0，且)(x f 在0x x =处有定义，则当=A 时，)(x f 在0x x =处连续.9、设)(x f 在[]1,0上有连续的导数且2)1(=f ，?=103)(dx x f ，则=1')(dx x xf10、设1=，⊥，则=+?)(b a a11、设x e u xysin =，=??xu12、=??Ddxdy . 其中D 为以点)0,0(O 、)0,1(A 、)2,0(B 为顶点的三⾓形区域.三、解答题（本⼤题共8⼩题，每⼩题8分，满分64分）13、计算11lim31--→x x x . 14、若函数)(x y y =是由参数⽅程-=+=tt y t x arctan )1ln(2所确定，求dx dy 、22dx y d .15、计算?+dx xxln 1. 16、计算dx x x ?20.17、求微分⽅程2'2y xy y x -=的通解.18、将函数)1ln()(x x f +=展开为x 的幂函数（要求指出收敛区间）. 19、求过点)2,1,3(-M 且与⼆平⾯07=-+-z y x 、0634=-+-z y x 都平⾏的直线⽅程.20、设),(2xy x xf z =其中),(v u f 的⼆阶偏导数存在，求y z ??、x y z 2.四、证明题（本题满分8分）.21、证明：当2≤x 时，233≤-x x .五、综合题（本⼤题共3⼩题，每⼩题10分，满分30分）22、已知曲线)(x f y =过原点且在点),(y x 处的切线斜率等于y x +2，求此曲线⽅程.第 9 页23、已知⼀平⾯图形由抛物线2x y =、82+-=x y 围成. （1）求此平⾯图形的⾯积；（2）求此平⾯图形绕y 轴旋转⼀周所得的旋转体的体积.24、设??=≠=??00)(1)(t a t dxdy x f t t g t D ，其中t D 是由t x =、t y =以及坐标轴围成的正⽅形区域，函数)(x f 连续. （1）求a 的值使得)(t g 连续；（2）求)('t g .2007年江苏省普通⾼校“专转本”统⼀考试⾼等数学⼀、单项选择题（本⼤题共6⼩题，每⼩题4分，满分24分.）1、若2)2(lim0=→x x f x ，则=∞→)21(lim x xf xA 、41B 、21C 、2D 、42、已知当0→x 时，)1ln(22x x +是x n sin 的⾼阶⽆穷⼩，⽽x n sin ⼜是x cos 1-的⾼阶⽆穷⼩，则正整数=nA 、1B 、2C 、3D 、43、设函数)3)(2)(1()(---=x x x x x f ，则⽅程0)('=x f 的实根个数为B 、2C 、3D 、4 4、设函数)(x f 的⼀个原函数为x 2sin ，则=?dx x f )2('A 、C x +4cosB 、C x +4cos 21C 、C x +4cos 2D 、C x +4sin5、设dt t x f x ?=212sin )(，则=)('x fA 、4sin xB 、2sin 2x xC 、2cos 2x xD 、4sin 2x x 6、下列级数收敛的是A 、∑∞=122n n n B 、∑∞=+11n n nC 、∑∞=-+1)1(1n n nD 、∑∞=-1)1(n n n。