2015 AMC美国数学竞赛试题及答案
AMC Intermediate 2015AMC-D:9-10年级中文历年真题

2015INTERMEDIATE DIVISIONAUSTRALIAN S CHOOL YEARS 9 and 10TIME ALLOWED: 75 MINUTESINSTRUCTIONS AND INFORMATIONGENERAL1. Do not open the booklet until told to do so by your teacher.2. NO calculators, maths stencils, mobile phones or other calculating aids are permitted. Scribbling paper, graphpaper, ruler and compasses are permitted, but are not essential. 3. Diagrams are NOT drawn to scale. They are intended only as aids.4. There are 25 multiple-choice questions, each with 5 possible answers given and 5 questions that require awhole number answer between 0 and 999. The questions generally get harder as you work through the paper. There is no penalty for an incorrect response.5. This is a competition not a test; do not expect to answer all questions. You are only competing against yourown year in your own country/Australian state so different years doing the same paper are not compared. 6. Read the instructions on the answer sheet carefully. Ensure your name, school name and school year areentered. It is your responsibility to correctly code your answer sheet. 7. When your teacher gives the signal, begin working on the problems.THE ANSWER SHEET 1. Use only lead pencil.2. Record your answers on the reverse of the answer sheet (not on the question paper) by FULLY colouring thecircle matching your answer.3. Your answer sheet will be scanned. The optical scanner will attempt to read all markings even if they are inthe wrong places, so please be careful not to doodle or write anything extra on the answer sheet. If you want to change an answer or remove any marks, use a plastic eraser and be sure to remove all marks and smudges.INTEGRITY OF THE COMPETITIONThe AMT reserves the right to re-examine students before deciding whether to grant official status to their score.©AMT P ublishing 2015 AMTT liMiTed Acn 083 950 341A ustrAliAn M AtheMAtics c oMpetitionsponsored by the c oMMonweAlth b AnkAn AcTiviTy of The AusTrAliAn MATheMATics TrusTNAMEYEA TEACHE RA u s T r A l i A n M A T h e M A T i c s T r u s TIntermediate Division Questions1to10,3marks each1.What is the area of this triangle in squarecentimetres?(A)10(B)12(C)14(D)7(E)62cm2.A movie lasts for213hours.The movie is shown in two equal sessions.For how many minutes does each session last?(A)85(B)70(C)80(D)65(E)753.If p=11and q=−4,then p2−q2equals(A)105(B)137(C)117(D)115(E)944.2015−20.15equals(A)1984.85(B)1995.15(C)1994.85(D)1995.85(E)2035.155.What is the value of2015twenty-cent coins?(A)$2015(B)$107.50(C)$17.50(D)$403(E)$436.Ana,Ben,Con,Dan and Eve are sitting around a ta-ble in that order.Ana calls out the number1,thenBen calls out the number2,then Con calls out thenumber3,and so on.After a person calls out a num-ber,the next person around the table calls out thenext number.Anyone who calls out a multiple of7must immediatelyleave the table.Who is the last person remaining at the table?(A)Ana(B)Ben(C)Con(D)Dan(E)Eve7.On a farm the ratio of horses to cows is3:2and ratio of cows to goats is4:3.The ratio of goats to horses is(A)5:7(B)3:8(C)3:5(D)5:18(E)1:28.Warren the window washer starts on the38thfloor of a building that has12windowsperfloor.He washes all of the windows on eachfloor before moving down to thefloor below.Whichfloor is Warren on after he has washed141windows?(A)25th(B)24th(C)28th(D)27th(E)26th9.A packet of lollies contains5blue lollies,15yellow lollies and some red lollies.One-third of the lollies are red.What fraction of the lollies are yellow?(A)13(B)56(C)12(D)16(E)2310.The diagram shows two small squares in opposite corners ofa large square.The squares have sides of length1cm,2cmand7cm.What is the area of the shaded pentagon?(A)18cm2(B)16cm2(C)22cm2(D)24cm2(E)20cm2Questions 11to 20,4marks each11.Jenna measures three sides of a rectangle and gets a total of 80cm.Dylan measuresthree sides of the same rectangle and gets a total of 88cm.What is the perimeter of the rectangle?(A)112cm(B)132cm(C)96cm(D)168cm(E)156cm12.A bar-tailed godwit was recorded by satellite tag in 2007tohave flown 11500km in eight days.On average,approximately how many kilometres per hour is that?(A)120(B)6(C)1(D)24(E)6013.A cube has the letters A,C,M,T,H and S on its six faces.Here are two views ofthis cube.CMAA MTWhich one of the following could be a third view of the same cube?(A)MHT(B)ATC(C)T SC(D)HTA (E)SCM 14.Two ordinary dice are rolled.The two resulting numbers are multiplied together tocreate a score.The probability of rolling a score that is a multiple of six is(A)16(B)512(C)14(D)13(E)1218.A strip of paper1cm wide is folded4times to make a regular octagon as shown.If the ends of the strip meet exactly when folded,how many centimetres long is the strip?√2(B)8(C)4+4√2(D)16(E)16−4√2(A)819.The country of Numismatica has six coins of thefollowing denominations:1cent,2cents,4cents, 10cents,20cents and40cents.Using the coins in my pocket,I can pay exactly for any amount up to and including200cents.What is the smallest number of coins I could have?(A)12(B)10(C)11(D)9(E)820.What fraction of the large triangle is shaded?(A)16(B)13(C)49(D)12(E)25Questions21to25,5marks each21.A student noticed that in a list offive integers,the mean,median and mode wereconsecutive integers in ascending order.What is the largest range possible for these five integers?(A)5(B)9(C)8(D)7(E)622.The square P QRS has sides of length2units andJ is the midpoint of P S.The line QJ intersectsthe diagonal P R at L.The length of LP is(A)√23(B)√33(C)√22(D)2√33(E)2√23SQ211PRJ23.For each integer from0to999,Andr´e wrote down the sum of its digits.What is theaverage of the numbers that Andr´e wrote down?(A)13.5(B)15(C)12(D)12.5(E)10.524.Max’s journey around this grid starts on a grid pointon side AB.He visits a grid point on each of sides BC,CD and DA in order before returning to his starting point, forming a quadrilateral.Max does not visit corner points A,B,C or D.How many journeys are possible which are not rect-angles?(Note that a square is a rectangle.)(A)256(B)252(C)64(D)248(E)76C B25.It takes Nicolai one and a half hours to paint the walls of a room and two hours topaint the ceiling.Elena needs exactly one hour to paint the walls of the same room and one hour to paint the ceiling.If Nicolai and Elena work together,what is the shortest possible time in minutes in which they can paint the walls and the ceiling of the room?(A)72(B)60(C)83(D)75(E)76For questions26to30,shade the answer as an integer from0to999in the space provided on the answer sheet.Question26is6marks,question27is7marks,question28is8marks, question29is9marks and question30is10marks.26.Mike has2015matches and uses them to builda triangular pattern like the one shown,but asbig as possible.How many matches does he haveleft over?27.How many positive integers n less than 2015have the property that 13+1ncan besimplified to a fraction with denominator less than n ?28.A rectangle has all sides of integer length.When 3units are added to the heightand 2units to the width,the area of the rectangle is tripled.What is the sum of the original areas of all such rectangles?29.At Berracan station,northbound trains arrive every three minutes starting at noonand finishing at midnight,while southbound trains arrive every five minutes starting at noon and finishing at midnight.Each day,I walk to Berracan station at a random time in the afternoon and wait for the first train in either direction.On average,how many seconds should I expect to wait?30.In a 14×18rectangle ABCD ,points P,Q,R and Sare chosen,one on each side of ABCD as pictured.The lengths AP ,P B ,BQ ,QC ,CR ,RD ,DS and SA are all positive integers and P QRS is a rectangle.What is the largest possible area that P QRS could have?DCB AQSIntermediate 2015 Answers Question Answer 1B2B3A4C5D6D7E8D9C10C11A12E13A14B15E16C17E18C19B20B21D22E23A24D25A2637272242844297430150。
美国数学学会中学生数学竞赛真题和答案解析2015AMC8 Solutions

This Solutions Pamphlet gives at least one solution for each problem on this year’s exam and shows that all the problems can be solved using material normally associated with the mathematics curriculum for students in eighth grade or below. These solutions are by no means the only ones possible, nor are they necessarily superior to others the reader may devise.We hope that teachers will share these solutions with their students. However, the publication, reproduction, or communication of the problems or solutions of the AMC 8 during the period when students are eligible to participate seriously jeopardizes the integrity of the results. Dissemination at any time via copier, telephone, email, internet or media of any type is a violation of the competition rules.Correspondence about the problems and solutions should be addressed to:Prof. Norbert Kuenzi, AMC 8 Chair934 Nicolet AveOshkosh, WI 54901-1634Orders for prior year exam questions and solutions pamphlets should be addressed to:MAA American Mathematics CompetitionsAttn: PublicationsPO Box 471Annapolis Junction, MD 20701© 2015 Mathematical Association of AmericaWe thank the following donors for their generous support of the MAA American Mathematics Competitions, MOSP and the IMOPatron’s CircleAkamai FoundationSimons FoundationWinner’s CircleAmerican Mathematical SocietyThe D.E. Shaw GroupDropboxMathWorksTwo SigmaTudor Investment CorporationAchiever’s CircleArt of Problem SolvingJane Street CapitalMath for AmericaSustainer’s circleAcademy of Applied ScienceArmy Educational Outreach ProgramCollaborator’s CircleAmerican Statistical AssociationCasualty Actuarial SocietyConference Board of the Mathematical SciencesExpii, Inc.IDEA MATHMu Alpha ThetaNational Council of Teachers of MathematicsSociety for Industrial and Applied MathematicsStar League。
2015年AMC12B竞赛真题及问题详解

2015 AMC 12B竞赛真题Problem 1What is the value of ?Problem 2Marie does three equally time-consuming tasks in a row without taking breaks. She begins the first task at 1:00 PM and finishes the second task at 2:40 PM. When does she finish the third task?Problem 3Isaac has written down one integer two times and another integer three times. The sum of the five numbers is 100, and one of the numbers is 28. What is the other number?Problem 4David, Hikmet, Jack, Marta, Rand, and Todd were in a 12-person race with 6 other people. Rand finished 6 places ahead of Hikmet. Marta finished 1 place behind Jack. David finished 2 places behind Hikmet. Jack finished 2 places behind Todd. Todd finished 1 place behind Rand. Marta finished in 6th place. Who finished in 8th place?Problem 5The Tigers beat the Sharks 2 out of the 3 times they played. They then played more times, and the Sharks ended up winning at least 95% of all the games played. What is the minimum possible value for ?Problem 6Back in 1930, Tillie had to memorize her multiplication facts fromto . The multiplication table she was given had rows and columns labeled with the factors, and the products formed the body of the table. To the nearest hundredth, what fraction of the numbers in the body of the table are odd?Problem 7A regular 15-gon has lines of symmetry, and the smallest positive angle for which it has rotational symmetry is degrees. What is ?Problem 8What is the value of ?Problem 9Larry and Julius are playing a game, taking turns throwing a ball at a bottle sitting on a ledge. Larry throws first. The winner is the first person to knock the bottle off the ledge. At each turn the probability that a playerknocks the bottle off the ledge is , independently of what has happened before. What is the probability that Larry wins the game?Problem 10How many noncongruent integer-sided triangles with positive area and perimeter less than 15 are neither equilateral, isosceles, nor right triangles?Problem 11The line forms a triangle with the coordinate axes. What is the sum of the lengths of the altitudes of this triangle?Problem 12Let , , and be three distinct one-digit numbers. What is the maximum value of the sum of the roots of the equation?Problem 13Quadrilateral is inscribed in a circle withand . What is ?Problem 14A circle of radius 2 is centered at . An equilateral triangle with side4 has a vertex at . What is the difference between the area of the regionthat lies inside the circle but outside the triangle and the area of the region that lies inside the triangle but outside the circle?Problem 15At Rachelle's school an A counts 4 points, a B 3 points, a C 2 points, and a D 1 point. Her GPA on the four classes she is taking is computed as the total sum of points divided by 4. She is certain that she will get As in both Mathematics and Science, and at least a C in each of English and History.She thinks she has a chance of getting an A in English, and a chance of getting a B. In History, she has a chance of getting an A, and a chanceof getting a B, independently of what she gets in English. What is the probability that Rachelle will get a GPA of at least 3.5?Problem 16A regular hexagon with sides of length 6 has an isosceles triangle attached to each side. Each of these triangles has two sides of length 8. The isosceles triangles are folded to make a pyramid with the hexagon as the base of the pyramid. What is the volume of the pyramid?Problem 17An unfair coin lands on heads with a probability of . When tossed times,the probability of exactly two heads is the same as the probability of exactly three heads. What is the value of ?Problem 18For every composite positive integer , define to be the sum of the factors in the prime factorization of . For example, because the prime factorization of is , and . What is the range of the function , ?Problem 19In , and . Squares and are constructed outside of the triangle. The points , , , and lie on a circle. What is the perimeter of the triangle?Problem 20For every positive integer , let be the remainder obtained when is divided by 5. Define a functionrecursively as follows:What is ?Problem 21Cozy the Cat and Dash the Dog are going up a staircase with a certain number of steps. However, instead of walking up the steps one at a time, both Cozy and Dash jump. Cozy goes two steps up with each jump (though if necessary, he will just jump the last step). Dash goes five steps up with each jump (though if necessary, he will just jump the last steps if there are fewer than 5 steps left). Suppose that Dash takes 19 fewer jumps than Cozy to reach the top of the staircase. Let denote the sum of all possible numbers of steps this staircase can have. What is the sum of the digits of ?Problem 22Six chairs are evenly spaced around a circular table. One person is seated in each chair. Each person gets up and sits down in a chair that is not the same chair and is not adjacent to the chair he or she originally occupied, so that again one person is seated in each chair. In how many ways can this be done?Problem 23A rectangular box measures , where , , and are integers and. The volume and the surface area of the box are numerically equal. How many ordered triples are possible?Problem 24Four circles, no two of which are congruent, have centers at , , , and , and points and lie on all four circles. The radius of circleis times the radius of circle , and the radius of circle is timesthe radius of circle . Furthermore, and . Let be the midpoint of . What is ?Problem 25A bee starts flying from point . She flies inch due east to point . For , once the bee reaches point , she turns counterclockwise and then flies inches straight to point . When the bee reaches she is exactly inches away from , where , , andare positive integers and and are not divisible by the square of any prime. What is ?2015 AMC 12B竞赛真题答案1.C2.b3.a4.b5.b6.a7.d8.d9.c 10.c 11.e 12.d 13.b 14.d 15.d 16.c 17.d 18.d 19.c 20.b 21.d 22.d 23.b 24.d 25.b。
2015年AMC8试题及答案

Copyright © 2016 Art of Problem Solving How many square yards of carpet are required to cover a rectangular floor that is feet long and feetwide? (There are 3 feet in a yard.)First, we multiply to get that you need square feet of carpet you need to cover. Since thereare square feet in a square yard, you divide by to get square yards, so our answer is .Since there are feet in a yard, we divide by to get , andby to get . To find the area of the carpet, we then multiply these two values together to get .2015 AMC 8 (Problems • Answer Key • Resources(/Forum/resources.php?c=182&cid=42&year=2015))Preceded byFirst Problem Followed by Problem 21 •2 •3 •4 •5 •6 •7 •8 •9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 •19 • 20 • 21• 22 • 23 • 24 • 25All AJHSME/AMC 8 Problems and SolutionsThe problems on this page are copyrighted by the Mathematical Association of America ()'s American Mathematics Competitions ().Placement:Easy GeometryRetrieved from "/wiki/index.php?title=2015_AMC_8_Problems/Problem_1&oldid=80323"SolutionSolution 2See AlsoPoint is the center of the regular octagon , and is the midpoint of the side What fraction of the area of the octagon is shaded?Since octagon is a regular octagon, it is split into equal parts, such as triangles, etc. These parts, since they are all equal, are of the octagon each. The shaded region consists of of these equal parts plus half of another, so the fraction of the octagon that is shaded isThe octagon has been divided up into identical triangles (and thus they each have equal area). Since the shaded region occupiesout of the total triangles, the answer is .For starters what I find helpful is to divide the whole octagon up into triangles as shown here:Now it is just a matter of counting the larger triangles remember that and are notfull triangles and are only half for these purposes. We count it up and we get a total ofof the shape shaded. We then simplify it to get our answer of .2015 AMC 8 (Problems • Answer Key • Resources(/Forum/resources.php?c=182&cid=42&year=2015))Preceded byProblem 1Followed by Problem 31 •2 •3 •4 •5 •6 •7 •8 •9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 •19 • 20 • 21 • 22 • 23 • 24 • 25All AJHSME/AMC 8 Problems and SolutionsSolution 3See AlsoCopyright © 2016 Art of Problem Solving Jack and Jill are going swimming at a pool that is one mile from their house. They leave home simultaneously. Jill rides her bicycle to the pool at a constant speed of miles per hour. Jack walks tothe pool at a constant speed of miles per hour. How many minutes before Jack does Jill arrive?Using , we can set up an equation for when Jill arrives at the swimming pool:Solving for , we get that Jill gets to the pool inof an hour, which is minutes. Doing the same for Jack, we get that Jack arrives at the pool inof an hour, which in turn is minutes. Thus, Jill has to waitminutes for Jack to arrive at the pool.2015 AMC 8 (Problems • Answer Key • Resources(/Forum/resources.php?c=182&cid=42&year=2015))Preceded byProblem 2Followed by Problem 41 •2 •3 •4 •5 •6 •7 •8 •9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 •19 • 20 • 21 • 22 • 23 • 24 • 25All AJHSME/AMC 8 Problems and Solutions The problems on this page are copyrighted by the Mathematical Association of America ()'s American Mathematics Competitions ().Retrieved from "/wiki/index.php?title=2015_AMC_8_Problems/Problem_3&oldid=81064"SolutionSee AlsoCopyright © 2016 Art of Problem SolvingThe Centerville Middle School chess team consists of two boys and three girls. A photographer wants to take a picture of the team to appear in the local newspaper. She decides to have them sit in a row with a boy ateach end and the three girls in the middle. How many such arrangements are possible?There are ways to order the boys on the end, and there are ways to order the girls in the middle.We get the answer to be .2015 AMC 8 (Problems • Answer Key • Resources(/Forum/resources.php?c=182&cid=42&year=2015))Preceded byProblem 3Followed by Problem 51 •2 •3 •4 •5 •6 •7 •8 •9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 •19 • 20 • 21 • 22 • 23 • 24 • 25All AJHSME/AMC 8 Problems and Solutions The problems on this page are copyrighted by the Mathematical Association of America ()'s American Mathematics Competitions ().Retrieved from "/wiki/index.php?title=2015_AMC_8_Problems/Problem_4&oldid=73224"SolutionSee AlsoCopyright © 2016 Art of Problem SolvingBilly's basketball team scored the following points over the course of the first 11 games of the season:If his team scores 40 in the 12th game, which of the following statistics will show an increase?When they score a on the next game, the range increases from to . This means the increased.Because is less than the score of every game they've played so far, the measures of center will neverrise. Only measures of spread, such as the, may increase.2015 AMC 8 (Problems • Answer Key • Resources(/Forum/resources.php?c=182&cid=42&year=2015))Preceded byProblem 4Followed by Problem 61 •2 •3 •4 •5 •6 •7 •8 •9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 •19 • 20 • 21 • 22• 23 • 24 • 25All AJHSME/AMC 8 Problems and SolutionsThe problems on this page are copyrighted by the Mathematical Association of America ()'s American Mathematics Competitions ().Retrieved from "/wiki/index.php?title=2015_AMC_8_Problems/Problem_5&oldid=80825"SolutionSolution 2Copyright © 2016 Art of Problem Solving In , , and . What is the area of?We know the semi-perimeter of is . Next, we use Heron's Formula to find that the area of the triangle is just .Splitting the isosceles triangle in half, we get a right triangle with hypotenuseand leg . Using the Pythagorean Theorem , we know the height is. Now that we know the height, the area is.2015 AMC 8 (Problems • Answer Key • Resources(/Forum/resources.php?c=182&cid=42&year=2015))Preceded byProblem 5Followed by Problem 71 •2 •3 •4 •5 •6 •7 •8 •9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 •19 • 20 • 21 • 22 • 23 •24 • 25All AJHSME/AMC 8 Problems and SolutionsThe problems on this page are copyrighted by the Mathematical Association of America ()'s American Mathematics Competitions ().Retrieved from "/wiki/index.php?title=2015_AMC_8_Problems/Problem_6&oldid=80483"Solution 1Solution 2See AlsoEach of two boxes contains three chips numbered , , . A chip is drawn randomly from each box and thenumbers on the two chips are multiplied. What is the probability that their product is even?We can instead find the probability that their product is odd, and subtract this from . In order to get an odd product, we have to draw an odd number from each box. We have a probability of drawing an odd numberfrom one box, so there is a probability of having an odd product. Thus, there is a probability of having an even product.You can also make this problem into a spinner problem. You have the first spinner with equally divided sections, andYou make a second spinner that is identical to the first, with equal sections of ,, and . If the first spinner lands on , to be even, it must land on two. You write down the first combination of numbers . Next, if the spinner lands on , it can land on any number on the second spinner. We now have the combinations of . Finally, if the first spinner ends on , we have Since there arepossible combinations, and we have evens, the final answer is.2015 AMC 8 (Problems • Answer Key • Resources(/Forum/resources.php?c=182&cid=42&year=2015))Preceded byProblem 6Followed by Problem 81 •2 •3 •4 •5 •6 •7 •8 •9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 •19 • 20 • 21 • 22 • 23 • 24 • 25All AJHSME/AMC 8 Problems and SolutionsThe problems on this page are copyrighted by the Mathematical Association of America ()'s American Mathematics Competitions ().Retrieved from "/wiki/index.php?title=2015_AMC_8_Problems/Problem_7&oldid=73737"SolutionSolution 2See AlsoCopyright © 2016 Art of Problem Solving What is the smallest whole number larger than the perimeter of any triangle with a side of length and aside of length ?We know from the triangle inequality that the last side, , fulfills . Adding to both sides of the inequality, we get , and becauseis the perimeter ofour triangle, is our answer.2015 AMC 8 (Problems • Answer Key • Resources(/Forum/resources.php?c=182&cid=42&year=2015))Preceded byProblem 7Followed by Problem 91 •2 •3 •4 •5 •6 •7 •8 •9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 •19 • 20 • 21 • 22 • 23• 24 • 25All AJHSME/AMC 8 Problems and SolutionsThe problems on this page are copyrighted by the Mathematical Association of America ()'s American Mathematics Competitions ().Retrieved from "/wiki/index.php?title=2015_AMC_8_Problems/Problem_8&oldid=78101"SolutionSee AlsoCopyright © 2016 Art of Problem Solving On her first day of work, Janabel sold one widget. On day two, she sold three widgets. On day three, she sold five widgets, and on each succeeding day, she sold two more widgets than she had sold on the previousday. How many widgets in total had Janabel sold after working days?The sum of is The sum is just the sum of the first odd integers, which is2015 AMC 8 (Problems • Answer Key • Resources(/Forum/resources.php?c=182&cid=42&year=2015))Preceded byProblem 8Followed by Problem 101 •2 •3 •4 •5 •6 •7 •8 •9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 •19 • 20 • 21 • 22 • 23 • 24 • 25All AJHSME/AMC 8 Problems and Solutions The problems on this page are copyrighted by the Mathematical Association of America ()'sAmerican Mathematics Competitions ().Retrieved from "/wiki/index.php?title=2015_AMC_8_Problems/Problem_9&oldid=79933"Solution 1Solution 2See AlsoCopyright © 2016 Art of Problem SolvingHow many integers betweenandhave four distinct digits?The question can be rephrased to "How many four-digit positive integers have four distinct digits?",since numbers between and are four-digit integers. There are choices for the first number, since it cannot be , there are only choices left for the second number since it must differ from the first, choices for the third number, since it must differ from the first two, and choices for the fourth number,since it must differ from all three. This means there are integersbetweenandwith four distinct digits.2015 AMC 8 (Problems • Answer Key • Resources(/Forum/resources.php?c=182&cid=42&year=2015))Preceded by Problem 9Followed by Problem 111 •2 •3 •4 •5 •6 •7 •8 •9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 •19 • 20 • 21 • 22• 23 • 24 • 25All AJHSME/AMC 8 Problems and SolutionsThe problems on this page are copyrighted by the Mathematical Association of America ()'sAmerican Mathematics Competitions ().Retrieved from "/wiki/index.php?title=2015_AMC_8_Problems/Problem_10&oldid=81128"Solution 1See AlsoCopyright © 2016 Art of Problem SolvingIn the small country of Mathland, all automobile license plates have four symbols. The first must be a vowel (A, E, I, O, or U), the second and third must be two different letters among the 21 non-vowels, and thefourth must be a digit (0 through 9). If the symbols are chosen at random subject to these conditions, whatis the probability that the plate will read "AMC8"?There is one favorable case, which is the license plate says "AMC8". We must now find how many total cases there are. There are choices for the first letter (since it must be a vowel), choices for the second letter (since it must be of consonants), choices for the third letter (since it must differ from the second letter), and choices for the number. This leads to total possible license plates. That means the probability of a license plate saying "AMC8" is.The probability of choosing A as the first letter is . The probability of choosing next is. Theprobability of choosing C as the third letter is(since there areother consonants to choose fromother then M). The probability of having as the last number is . We multiply all these to obtain2015 AMC 8 (Problems • Answer Key • Resources(/Forum/resources.php?c=182&cid=42&year=2015))Preceded by Problem 10Followed by Problem 121 •2 •3 •4 •5 •6 •7 •8 •9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 •19 • 20 • 21 • 22 • 23 •24 • 25All AJHSME/AMC 8 Problems and SolutionsThe problems on this page are copyrighted by the Mathematical Association of America ()'sAmerican Mathematics Competitions ().Retrieved from "/wiki/index.php?title=2015_AMC_8_Problems/Problem_11&oldid=73554"Solution 1Solution 2See AlsoHow many pairs of parallel edges, such asandorand, does a cube have?We first count the number of pairs of parallel lines that are in the same direction as. The pairs ofparallel lines are ,, , , ,and . These are pairs total. We can do the same for the lines in the same direction asand. This means there aretotal pairs of parallel lines.Pick a random edge. Given another edge, the probability that it is parallel to this edge is. Keep in mind we already used one edge. There are edges so pairs. So our answer is.2015 AMC 8 (Problems • Answer Key • Resources(/Forum/resources.php?c=182&cid=42&year=2015))Preceded by Problem 11Followed by Problem 131 •2 •3 •4 •5 •6 •7 •8 •9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 •19 • 20 • 21 • 22 • 23 • 24 • 25All AJHSME/AMC 8 Problems and SolutionsSolution 1Solution 2See AlsoCopyright © 2016 Art of Problem SolvingHow many subsets of two elements can be removed from the set so thatthe mean (average) of the remaining numbers is 6?Since there will be elements after removal, and their mean is , we know their sum is . We also knowthat the sum of the set pre-removal is . Thus, the sum of the elements removed is .There are onlysubsets of elements that sum to:.2015 AMC 8 (Problems • Answer Key • Resources(/Forum/resources.php?c=182&cid=42&year=2015))Preceded by Problem 12Followed by Problem 141 •2 •3 •4 •5 •6 •7 •8 •9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 •19 • 20 • 21 • 22• 23 • 24 • 25All AJHSME/AMC 8 Problems and SolutionsThe problems on this page are copyrighted by the Mathematical Association of America ()'sAmerican Mathematics Competitions ().Retrieved from "/wiki/index.php?title=2015_AMC_8_Problems/Problem_13&oldid=73377"SolutionSee AlsoLet our numbers be , where is odd. Then our sum is . The only answer choice that cannot be written as , where is odd, is .If the four consecutive odd integers are and then the sum is . All the integers are divisible by except .If the four consecutive odd integers are and , the sum is , and divided by gives . This means that must be even. The only integer that does notgive an even integer when divided by is , so the answer is .From Solution 1, we have the sum of the numbers to be equal to . Taking mod 8 gives usfor some residue and for some odd integer . Since , we can express it as the equation for some integer . Multiplying 4 to each side of the equation yields , and taking mod 8 gets us , so . All the answer choicesexcept choice D is a multiple of 8, and since 100 satisfies all the conditions the answer is .The problems on this page are copyrighted by the Mathematical Association of America ()'s American Mathematics Competitions ().Copyright © 2016 Art of Problem SolvingAt Euler Middle School,students voted on two issues in a school referendum with the following results:voted in favor of the first issue and voted in favor of the second issue. If there were exactlystudents who voted against both issues, how many students voted in favor of both issues?We can see that this is a Venn Diagram Problem.First, we analyze the information given. There are students. Let's use A as the first issue and B asthe second issue.students were for the A, and students were for B. There were also students against both A andB.Solving this without a Venn Diagram, we subtract away from the total,. Out of the remaining,we havepeople for A andpeople for B. We add this up to get. Since that is more than what we need, we subtract fromto getThere are 198 people. We know that 29 people voted against both the first issue and the second issue. That leaves us with 169 people that voted for at least one of them. If 119 people voted for both of them, then that would leave 20 people out of the vote, because 149 is less than 198 people. 198-149 is 20, so to make it even, we have to take 20 away from the 119 people, which leaves us with2015 AMC 8 (Problems • Answer Key • Resources(/Forum/resources.php?c=182&cid=42&year=2015))Preceded by Problem 14Followed by Problem 161 •2 •3 •4 •5 •6 •7 •8 •9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 •19 • 20 • 21 • 22• 23 • 24 • 25All AJHSME/AMC 8 Problems and SolutionsThe problems on this page are copyrighted by the Mathematical Association of America ()'sAmerican Mathematics Competitions ().Retrieved from "/wiki/index.php?title=2015_AMC_8_Problems/Problem_15&oldid=80999"Solution 1Solution 2See AlsoCopyright © 2016 Art of Problem SolvingIn a middle-school mentoring program, a number of the sixth graders are paired with a ninth-grade student as a buddy. No ninth grader is assigned more than one sixth-grade buddy. If of all the ninth graders are paired withof all the sixth graders, what fraction of the total number of sixth and ninth graders have a buddy?Let the number of sixth graders be , and the number of ninth graders be . Thus, , whichsimplifies to. Since we are trying to find the value of, we can just substitute forinto the equation. We then get a value ofWe see that the minimum number of ninth graders is , because if there arethen there is ninth grader with a buddy, which would mean sixth graders with a buddy, and that's impossible. With ninth graders, of them are in the buddy program, so theresixth graders total, two of whom have a buddy. Thus,the desired fraction is .2015 AMC 8 (Problems • Answer Key • Resources(/Forum/resources.php?c=182&cid=42&year=2015))Preceded by Problem 15Followed by Problem 171 •2 •3 •4 •5 •6 •7 •8 •9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 •19 • 20 • 21 • 22• 23 • 24 • 25All AJHSME/AMC 8 Problems and SolutionsThe problems on this page are copyrighted by the Mathematical Association of America ()'sAmerican Mathematics Competitions ().Retrieved from "/wiki/index.php?title=2015_AMC_8_Problems/Problem_16&oldid=73512"Solution 1Solution 2See AlsoSo and .This gives , which gives , which then givesSolution 2, is obviously constantso , plug into the first one and it's miles to schoolWe set up an equation in terms of the distance and the speed In miles per hour. We have SoAn arithmetic sequence is a sequence in which each term after the first is obtained by adding a constant to the previous term. For example, is an arithmetic sequence with five terms, in which thefirst term is and the constant added is . Each row and each column in this array is an arithmetic sequence with five terms. What is the value of ?We begin filling in the table. The top row has a first term and a fifth term , so we have the common difference is . This means we can fill in the first row of the table:The fifth row has a first term of and a fifth term of , so the common difference is. We can fill in the fifth row of the table as shown:Copyright © 2016 Art of Problem SolvingWe must find the third term of the arithmetic sequence with a first term of and a fifth term of . The common difference of this sequence is, so the third term is.The middle term of the first row is, since the middle number is just the average in anarithmetic sequence. Similarly, the middle of the bottom row is . Applying this again forthe middle column, the answer is.The value ofis simply the average of the average values of both diagonals that contain. This is2015 AMC 8 (Problems • Answer Key • Resources(/Forum/resources.php?c=182&cid=42&year=2015))Preceded by Problem 17Followed by Problem 191 •2 •3 •4 •5 •6 •7 •8 •9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 •19 • 20 • 21 • 22 • 23 • 24• 25All AJHSME/AMC 8 Problems and SolutionsThe problems on this page are copyrighted by the Mathematical Association of America ()'sAmerican Mathematics Competitions ().Retrieved from "/wiki/index.php?title=2015_AMC_8_Problems/Problem_18&oldid=73460"Solution 2Solution 3See AlsoA triangle with vertices as , , and is plotted on a grid. What fraction of the grid is covered by the triangle?The area of is equal to half the product of its base and height. By the Pythagorean Theorem, we find its height is , and its base is . We multiply these and divideby to find the of the triangle is . Since the grid has an area of , the fraction of the grid covered by the triangle is .Note angle is right, thus the area is thus the fraction of the total isCopyright © 2016 Art of Problem SolvingBy the Shoelace theorem, the area of.This means the fraction of the total area isThe smallest rectangle that follows the grid lines and completely encloses has an area of,where splits the rectangle into four triangles. The area ofis therefore . That means thattakes upof the grid.Using Pick's Theorem, the area of the triangle is. Therefore, the triangle takes upof the grid.2015 AMC 8 (Problems • Answer Key • Resources(/Forum/resources.php?c=182&cid=42&year=2015))Preceded by Problem 18Followed by Problem 201 •2 •3 •4 •5 •6 •7 •8 •9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 •19 • 20 • 21 • 22• 23 • 24 • 25All AJHSME/AMC 8 Problems and SolutionsThe problems on this page are copyrighted by the Mathematical Association of America ()'sAmerican Mathematics Competitions ().Retrieved from "/wiki/index.php?title=2015_AMC_8_Problems/Problem_19&oldid=75879"Solution 4Solution 5See AlsoRalph went to the store and bought 12 pairs of socks for a total of $24. Some of the socks he bought cost $1a pair, some of the socks he bought cost $3 a pair, and some of the socks he bought cost $4 a pair. If hebought at least one pair of each type, how many pairs of $1 socks did Ralph buy?So let there be pairs of socks, pairs ofsocks, pairs of socks.We have,, and.Now we subtract to find , and . It follows that is a multiple of and is amultiple of , so since , we must have .Therefore, , and it follows that. Now, asdesired.Since the total cost of the socks was and Ralph boughtpairs, the average cost of each pair ofsocks is.There are two ways to make packages of socks that average to . You can have:Twopairs and one pair (package adds up to )Onepair and onepair (package adds up to)So now we need to solvewhere is the number of packages and is the number of packages. We see our only solution (thathas at least one of each pair of sock) is , which yields the answer of.2015 AMC 8 (Problems • Answer Key • Resources(/Forum/resources.php?c=182&cid=42&year=2015))Preceded by Problem 19Followed by Problem 211 •2 •3 •4 •5 •6 •7 •8 •9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 •19• 20 • 21 • 22 • 23 • 24 • 25All AJHSME/AMC 8 Problems and SolutionsThe problems on this page are copyrighted by the Mathematical Association of America ()'sAmerican Mathematics Competitions ().Retrieved from "/wiki/index.php?2015 AMC 8 Problems/Problem 20Solution 1Solution 2See AlsoIn the given figure hexagon is equiangular, andare squares with areasandrespectively, is equilateral and . What is the area of ?.Clearly, since is a side of a square with area , . Now, since , we have .Now, is a side of a square with area, so . Since is equilateral, . Lastly, is a right triangle. We see that, so is a right triangle with legs and . Now, its area is .2015 AMC 8 (Problems • Answer Key • Resources(/Forum/resources.php?c=182&cid=42&year=2015))Preceded byProblem 20Followed byProblem 221 •2 •3 •4 •5 •6 •7 •8 •9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 •21•22 • 23 • 24 • 25All AJHSME/AMC 8 Problems and SolutionsThe problems on this page are copyrighted by the Mathematical Association of America ()'s American Mathematics Competitions ().Retrieved from "/wiki/index.php?title=2015_AMC_8_Problems/Problem_21&oldid=73361"2015 AMC 8 Problems/Problem 21SolutionSee AlsoCopyright © 2016 Art of Problem SolvingCopyright © 2016 Art of Problem SolvingOn June 1, a group of students is standing in rows, with 15 students in each row. On June 2, the same group is standing with all of the students in one long row. On June 3, the same group is standing with just one student in each row. On June 4, the same group is standing with 6 students in each row. This processcontinues through June 12 with a different number of students per row each day. However, on June 13, they cannot find a new way of organizing the students. What is the smallest possible number of students in thegroup?As we read through this text, we find that the given information means that the number of students in thegroup has factors, since each arrangement is a factor. The smallest integer with factors is.2015 AMC 8 (Problems • Answer Key • Resources(/Forum/resources.php?c=182&cid=42&year=2015))Preceded by Problem 21Followed by Problem 231 •2 •3 •4 •5 •6 •7 •8 •9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 •19 • 20 • 21 • 22 • 23 • 24 •25All AJHSME/AMC 8 Problems and SolutionsThe problems on this page are copyrighted by the Mathematical Association of America ()'sAmerican Mathematics Competitions ().Retrieved from "/wiki/index.php?title=2015_AMC_8_Problems/Problem_22&oldid=73359"SolutionSee Also。
AMC10A 2015 题目
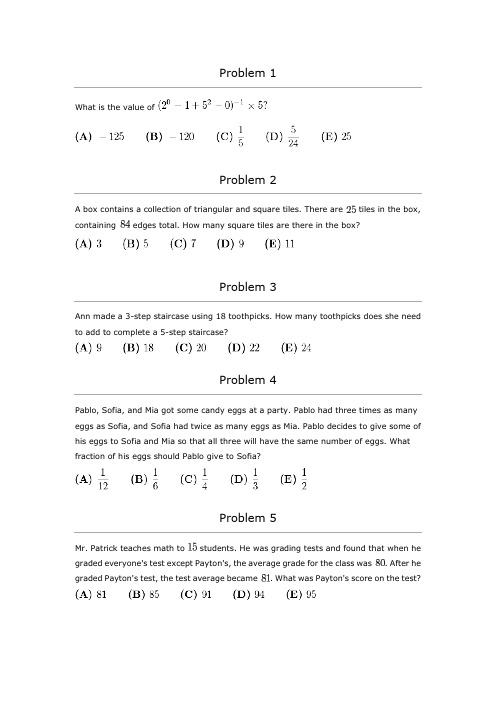
Problem 1What is the value ofProblem 2A box contains a collection of triangular and square tiles. There are tiles in the box,containing edges total. How many square tiles are there in the box?Problem 3Ann made a 3-step staircase using 18 toothpicks. How many toothpicks does she need to add to complete a 5-step staircase?Problem 4Pablo, Sofia, and Mia got some candy eggs at a party. Pablo had three times as many eggs as Sofia, and Sofia had twice as many eggs as Mia. Pablo decides to give some of his eggs to Sofia and Mia so that all three will have the same number of eggs. What fraction of his eggs should Pablo give to Sofia?Problem 5Mr. Patrick teaches math to students. He was grading tests and found that when he graded everyone's test except Payton's, the average grade for the class was . After hegraded Payton's test, the test average became . What was Payton's score on the test?Problem 6The sum of two positive numbers is times their difference. What is the ratio of the larger number to the smaller number?Problem 7How many terms are there in the arithmetic sequence , , , . . ., , ?problem 8Two years ago Pete was three times as old as his cousin Claire. Two years before that, Pete was four times as old as Claire. In how many years will the ratio of their agesbe : ?problem 10How many rearrangements of are there in which no two adjacent letters are also adjacent letters in the alphabet? For example, no such rearrangements could include either or .problem 11The ratio of the length to the width of a rectangle is : . If the rectangle has diagonalof length , then the area may be expressed as for some constant . What is ?Problem 12Points and are distinct points on the graph of .What is ?Problem 13Claudia has 12 coins, each of which is a 5-cent coin or a 10-cent coin. There are exactly 17 different values that can be obtained as combinations of one or more of her coins. How many 10-cent coins does Claudia have?Problem 15Consider the set of all fractions where and are relatively prime positive integers. How many of these fractions have the property that if both numerator and denominatorare increased by , the value of the fraction is increased by ?Problem 16If , and , what is the value of ?Problem 18Hexadecimal (base-16) numbers are written using numeric digits through as well asthe letters through to represent through . Among the first positiveintegers, there are whose hexadecimal representation contains only numeric digits. What is the sum of the digits of ?Problem 19The isosceles right triangle has right angle at and area . The raystrisecting intersect at and . What is the area of ?Problem 20A rectangle has area and perimeter , where and are positive integers.Which of the following numbers cannot equal ?Problem 21Tetrahedron has , , , , ,and . What is the volume of the tetrahedron?Problem 22Eight people are sitting around a circular table, each holding a fair coin. All eight people flip their coins and those who flip heads stand while those who flip tails remain seated. What is the probability that no two adjacent people will stand?Problem 23The zeros of the function are integers. What is the sum of thepossible values of ?Problem 24For some positive integers , there is a quadrilateral with positive integer side lengths, perimeter , right angles at and , , and . How manydifferent values of are possible?Problem 25Let be a square of side length . Two points are chosen independently at random on the sides of . The probability that the straight-line distance between the points is atleast is , where , , and are positive integers with . Whatis ? ( gcd 最大公约数)。
2015年美国“数学大联盟杯赛”(中国赛区)初赛六年级试卷

37. In this problem as shown on the right, each square represents a digit from 0 to 9. What is the final product? Answer: ___________. 38. For some integer n, the sum of all of the digits of n is a multiple of 8 and the sum of all of the digits of n + 1 is also a multiple of 8. If n <= 2015, what is the greatest possible value of n? Answer: ___________. 39. In triangle ABC on the right, BD:DC = 1:2. If the area of triangle ABP is 7 and the area of triangle ACP is 5, what is the area of triangle ADP? 2 0 1 5 A P
性别
年级
1. 2 × 0 + 1 × 4 – 2 × 0 + 1 × 5 = ( ) A) 0 B) 5 C) 9 D) 15 2. There are 84 beavers in a colony. Yesterday they all went out in teams of 6 to collect logs. Today they are all going out in teams of 4. There will be ? more teams today than there were yesterday. ( ) A) 2 B) 7 C) 14 D) 21 3. 12 345 + 54 321 = 11 111 × ? A) 66 666 B) 666 ( ) C) 66 D) 6 ? years mmon multiple of 22, 42, 62, 82, and 102 is ( ) A) 3840 B) 14 400 C) 57 600 D) 230 400 28. A snail crawls 2400 mm per hour. It takes ? seconds to crawl 1 mm. ( ) 1 2 A) B) C) 1.5 D) 3 3 3 29. A circle can intersect a quadrilateral at ? points. ( ) A) 0, 2 or 8 only B) 0, 2, 4, 6 or 8 only C) 1, 2, 3, 4, 6, or 8 only D) 0, 1, 2, 3, 4, 5, 6, 7, or 8 30. Carla loves shells! She collects them, labelling each with a 1-, 2-, or 3-letter code. If the same letters are used in a different order, it is a different code. She has used every such code, so she has ? shells. ( ) A) 156 B) 17 576 C) 18 278 D) 20 888 31. If the sum of 50 consecutive integers is 1525, what is the sum of the next 50 consecutive integers? ( ) A) 1575 B) 2775 C) 4025 D) 76 250 32. Of 4 pairs of twins, 3 students are chosen to do a report together. There are ? possible groups of 3 that don’t include both twins from a pair. ( ) A) 16 B) 24 C) 28 D) 32 33. 八年后我的年龄是两年前我的年龄的两倍。请问两年后我是几岁? ( ) A) 14 B) 16 C) 18 D) 20 34. 将 0.75 转换为分数,这些分数的分子和分母都是小于 100 的正整数。这样的分数共有 多少个? ( ) A) 3 B) 24 C) 25 D) 33 35. 船长们划船 5 个小时。从第二个小时开始,每个小时行驶的距离比 前一个小时少了 2 千米。如果 5 个小时的平均行驶速度是 6 千米/小时,那么前 2 个小时行驶了多少千米? ( ) A) 6 B) 12 C) 16 D) 18 二、填空题(每小题 5 分,答对加 5 分,答错不扣分,共 25 分) 36. Calculate the number of digits which are equal to 0 in the product of 826446281, 11 and 11. Answer: ___________.
2015年美赛数学建模题目

2015 Contest ProblemsMCM PROBLEMSPROBLEM A: Eradicating EbolaThe world medical association has announced that their new medication could stop Ebola and cure patients whose disease is not advanced. Build a realistic, sensible, and useful model that considers not only the spread of the disease, the quantity of the medicine needed, possible feasible delivery systems, locations of delivery, speed of manufacturing of the vaccine or drug, but also any other critical factors your team considers necessary as part of the model to optimize the eradication of Ebola, or at least its current strain. In addition to your modeling approach for the contest, prepare a 1-2 page non-technical letter for the world medical association to use in their announcement.PROBLEM B: Searching for a lost planeRecall the lost Malaysian flight MH370. Build a generic mathematical model that could assist "searchers" in planning a useful search for a lost plane feared to have crashed in open water such as the Atlantic, Pacific, Indian, Southern, or Arctic Ocean while flying from Point A to Point B. Assume that there are no signals from the downed plane. Your model should recognize that there are many different types of planes for which we might be searching and that there are many different types of search planes, often using different electronics or sensors. Additionally, prepare a 1-2 page non-technical paper for the airlines to use in their press conferences concerning their plan for future searches.ICM PROBLEMSPROBLEM C: Managing Human Capital in OrganizationsClick the title below to download a PDF of the 2015 ICM Problem C.Your ICM submission should consist of a 1 page Summary Sheet and your solution cannot exceed 20 pages for a maximum of 21 pages.Managing Human Capital in OrganizationsPROBLEM D: Is it sustainable?Click the title below to download a PDF of the 2015 ICM Problem D.Your ICM submission should consist of a 1 page Summary Sheet and your solution cannot exceed 20 pages for a maximum of 21 pages.Is it sustainable?。
2015年美国数学建模竞赛第二次模拟赛题c题

Prblem C Forest FiresOne major environmental concern is the occurrence of forest fires (also called wildfires), which affect forest preservation, bring economical and ecological damage and endanger human lives. Such phenomenon is due to multiple causes (e.g. human negligence and lightnings). Despite an increasing of state expenses to control this disaster, each year millions of forest hectares (ha) are destroyed all around the world.Fast detection is an important element for successful firefighting. Traditional human surveillance is expensive and affected by subjective factors, there has been an emphasis to develop automatic solutions, such as satellite-based, infrared/smoke scanners and local sensors (e.g. meteorological). Propagation models try to describe the future evolution of the forest fire given an initial scenario and certain input parameters. Modeling the dynamical behavior of fire propagation in a forest is helpful for creating scheme to control and fight fire.Requirement 1Describe several different metrics that could be used to evaluate the effectiveness of fire detection. Could you combine your metrics to make them even more useful for measuring quality?Requirement 2Model the dynamical behavior of fire spread in a forest.Requirement 3 Discuss the factors to affect fire occurrence. Which factors are the most critical in causing fires. Build mathematical models to predict the burned area of fires using Meteorological Data.Requirement 4 Give y our suggestion for preventing from forest fire and fighting against it.。
2015AMC10A试题及详解(繁体中文版)

2
h 1 1.1 r 2ຫໍສະໝຸດ h 2 h 1 1.21 h 2
10. 將四個字母 abcd 重新排列,但原來相鄰的字母都不能再排相鄰(例如: ab 或 ba 都不能 出現在重新的排列中),試問共有幾種排法? (A) 0 (B)1 (C) 2 (D) 3 (E) 4 。 【2015AMC10A】
19. 等腰直角 ABC 中, ACB 為直角且面積為 12.5 ,若 ACB 的三等分角線分別交 AB 於 D 、 E 兩點,則 CDE 的面積是多少? (A)
5 3 2
(B)
50
3 75 4
2
(C)
15 3 8
(D)
50 25 3 2
(E)
25 。 6
【2015AMC10A】
【2015AMC10A】
答: (C) 解: 圓盤中心到鐘面圓心距離為 20 10 30 故圓盤繞鏡面滾回原處時,箭頭共有 3 次指向正上方,第一次為
1 圈( 4 : 00 )處 3
15. 考慮由分數
x 組成的集合,其中 x 與 y 是互質的正整數,試問在此集合中有多少個分數 y 滿足:當分子與分母各加 1 時,新分數比原來的分數增加了 10% ? (A) 0 (B)1 (C) 2 (D) 3 (E)無限多。 【2015AMC10A】
2
11. 某個長方形長與寬的比為 4 : 3 ,若此長方形對角線的長為 d ,且其面積等於 kd , 則 k 之值為何? 2 3 12 16 3 (A) (B) (C) (D) (E) 。 【2015AMC10A】 7 7 25 25 4 答: (C) 解: 長 4 t ,寬 3 t ,對角線 d 5 t
(参考资料)美国数学大联盟2015年复赛试题

2015-2016 Math League Contests, Grades 9 – 12Second-Round, Jan - Feb 2016Instructions:1.This second-round contest consists of two parts. Part 1 is math questions. Part 2 is essay.2.For all the questions below, login to your account at / , and enter youranswers. Answers written on this document will NOT be credited.3.In Part 1, you are asked to read one math subject, The Mathematics of Normal Distributions, and thesupplementary materials if necessary, Descriptive Statistics. Then you have 28 questions to work on.You will need to give precise, unambiguous answers to Questions 1-19 (The Mathematics of Normal Distributions), and Questions 21-26 (Descriptive Statistics).4.Question 20 (The Mathematics of Normal Distributions) and 27-28 (Descriptive Statistics) areProjects and Papers, which means you need to do your research and write a paper for each question.There is no word limit on each of your papers, but it doesn’t necessary mean the more words thebetter. The best paper is precise and succinct. Please don’t feel frustrated at all if you can’t write apaper, as the topics, Confidence Intervals (Question 20); Lies, Damn Lies, and Statistics (Question27); and Data in Your Daily Life (Question 28), are very hard for a high school student, even for anadult. Please don’t feel frustrated even if you can’t finish all of Questions 1-19, 21-26, as they are not trivial questions and it requires a lot reading and thinking. Students who can work out a few questions should be commended.5.For the subject of Normal Distributions, if you really understand what it is and how it works,then the questions are fairly easy to solve. So our recommendation is don’t rush to solve theproblems. Instead please take your time to read and understand the subject thoroughly. Once you understand how Normal Distributions works, you can solve most of the problems without much difficulty. So this is really a test of your research and analytical skill, your patience, and perseverance.6.In order to understand Normal Distributions, you need to be familiar with basic statistics termssuch as mean, median, standard deviation, percentile, quartile, and etc. It is a good idea to read the subject Descriptive Statistics, the supplementary materials, thoroughly to refresh yourmemory.7.The more questions you answered correctly, the more credit you will get. The total credit, or perfectscore, of Part 1 is 180. The total credit, or perfect score, of Part 2, is 90. The problems are ordered by content, NOT DIFFICULTY. It is to your advantage to attempt problems from throughout the test. 8.You can seek help by reading books, searching the Internet, asking an expert, and etc. But you can’tdelegate this to someone else and turn in whatever he/she wrote for you. To make it clear, the purpose of the second-round contest is to test your ability to read and research. You need to be the one who understand the topics and solve the problems. You will be caught if it is not the case during theinterview.9.For Part 1, you can write in either English or Chinese.10.In Part 2, you are asked to write an essay. You have to write in English in Part 2.11.If you have any questions regarding the contest, please contact us at once atINFO@12.This document contains 16 pages in total, including this page.13.Submission of your answers:a)For all the questions below, login to your account at / , and enter youranswers. Answers written on this document will NOT be credited.b)You need to submit your answers no later than 12:00AM, Feb 7, 2016, Beijing Time. Latersubmission will not be accepted.Part 1 – The Mathematics of Normal DistributionThe following is an excerpt from some math book.The Mathematics of Normal Distributions, (see separate document).Understanding the above Chapter on “Normal Distributions” requires familiarity with basic statistics terms such as mean, median, standard deviation, percentile, quartile, and etc. It helps to read the following Chapter on “Descriptive Statistics”, the supplementary materials, if you need to refresh your memory of these terms.Descriptive Statistics, (the supplementary materials, see separate document).For all the questions below, login to your account at / , and enter your answers. Answers written on this document will NOT be credited.Question 1: (credit: 4)Hint:1Q is the first quartile.3Q is the third quartile. For the definition of 1Q and 3Q , see Section 14.3, of Chapter 14, “Descriptive Statistics.”Question 2: (credit: 4)Estimate the value of the standard deviation (rounded to the nearest inch) of a normal distribution with 81.2μ=inch and 394.7Q =inch.Hint:3Q is the third quartile. For the definition of 3Q , see Section 14.3, of Chapter 14, “Descriptive Statistics.”Question 3: (credit: 4)Note:1Q is the first quartile.3Q is the third quartile. For the definition of 1Q and 3Q , see Section 14.3, of Chapter 14, “Descriptive Statistics.”Note: For this question, please write your answer on file “high-school-answersheet.doc ”, downloadable together with this document at , and submit file “high-school-answersheet.doc ” at after you are done.Question 4: (credit: 4)Question 5: (credit: 4)Question 6: (credit: 4): Question 7: (credit: 4)Question 8: (credit: 4)Question 9: (credit: 4)Questions 10 & 11 refer to the following:Note: For the definition of percentile, see Section 14.3, of Chapter 14, “Descriptive Statistics.”Question 10: (credit: 6)Question 11: (credit: 6)Hint:(c) Rounded to the nearest pound.Question 12: (credit: 6)Hint:(b)Rounded to nearest integer.(c)Enter your answer as a decimal between 0 and 1, rounded to the nearest hundredth. Question 13: (credit: 6)Hint:(b)Rounded to nearest thousandth.(c)Rounded to the nearest hundredth.Question 14: (credit: 4)Questions 15-18:In Questions 15-18, you should use the table above to make your estimates.Note: For the definition of percentile, s ee Section 14.3, of Chapter 14, “Descriptive Statistics.”Question 15: (credit: 4)Question 16: (credit: 4)Consider again the distribution of weights of six-month-old baby boys in Question 15.Question 17: (credit: 6)Question 18: (credit: 8)Question 19: (credit: 4)Question 20: (credit: 20, note: paper with exceptional quality can get up to 40 credits)Note: You can write in either Engish or Chinese.Note: For this question, please write your answer on file “high-school-answersheet.doc”, downloadable together with this document at , and submit file “high-school-answersheet.doc” at after you are done.Question 21: (credit: 4)Question 22: (credit: 4)The two histograms below summarize the team payrolls in Major League Baseball (2008).Using the information in the figure, where did the median payroll of 2008 baseball teams fall?(a)Somewhere between $50 million and $80 million(b)Somewhere between $70 million and $80 million(c)Somewhere between $70 million and $100 million(d)Somewhere between $80 million and $100 millionQuestion 23: (credit: 6)This question refers to histograms with unequal class intervals.Question 24: (credit: 8)Note: For (c) and (d), please write your answer on file “high-school-answersheet.doc ”, downloadable together with this document at , and submit file “high-school-answersheet.doc ” at after you are done.Question 25: (credit: 4)Let A denote the mean of data set 12{,,...,}N x x x . Let B denote the mean of dataset 12{,,...,}N x c x c x c +++.(a) Find the relationship between A and B .a) A B <b) A B c =-c) A B c =+d) Nondeterministic(b) Find the mean of 12{,,...,}N x A x A x A ---.Question 26: (credit: 4)Let 1R and 1σdenote the range and standard deviation of data set 12{,,...,}N x x x , respectively. Let 2R and 2σdenote the range and standard deviation of data set 12{,,...,}N x c x c x c +++, respectively. (a) Find the relationship between 1R and 2R .a) Nondeterministicb) 1R = 2Rc) 1R = 2R + cd) 1R = 2R - c(b) Find the relationship between 1σand 2σ.a) Nondeterministicb) 1σ = 2σc) 1σ = 2σ+ cd) 1σ = 2σ- cQuestion 27: (credit: 20, note: paper with exceptional quality can get up to 40 credits)Note: You can write in either Engish or Chinese.Note: For this question, please write your answer on fi le “high -school-answersheet .doc”, downloadable together with this document at , and submit file“high-school-answersheet.doc” at after you are done.Question 28: (credit: 20, note: paper with exceptional quality can get up to 40 credits)Note: You can write in either Engish or Chinese.Note: For this question, please write your answer on fi le “high-school-answersheet.do c”, downloadable together with this document at , and submit file “high-school-answersheet.doc” at after you are done.Part 2 Essay (Credit: 90)Pros and Cons of Math Competitionsby Richard RusczykMathematics competitions such as MATH LEAGUE, MATHCOUNTS, and the American Mathematics Competitions are probably the extracurricular math programs with the widest participation. The most immediate value of these math contests is obvious –they pique students’ interest in mathematics and encourage them to value intellectual pursuits. Kids love games, and many will turn just about any activity into a contest, or in other words, something to get good at. Math contests thus inspire them to become good at mathematics just like sports encourage physical fitness. Eventually, students put aside the games. By then, hopefully an interest in the underlying activity has developed.Beyond encouraging an interest in mathematics, contests help prepare students for competition. For better or worse, much of life is competition, be it for jobs or resources or whatever. Competition of any sort trains students to deal with success and failure, and teaches them that effective performance requires practice. Moreover, nearly every interesting and worthwhile venture in life comes with some element of pressure; competition teaches students how to handle it.Despite all the benefits of math contests, they are not an unmitigated good. First of all, not all contests are designed well. Students shouldn’t take too seriously contests that greatly emphasize speed or memorization. Curricular contests (particularly calculus contests for high school students) can also be misleading, as they deepen the misconception that there is no more to math than what is in the classroom. Such contests run the risk of encouraging students to overvalue skills that aren’t nearly as valuable as the one asset a contest should help them develop – the ability to think about and solve complex problems.A second danger of contests is extending kids beyond their ability. Students should certainly be challenged with problems they can’t do from time to time, but if this happens consistently, the experien ce goes from humbling and challenging to humiliating and discouraging.A third potential pitfall, burnout, often comes on the heels of the first two. Participants in math contests are just as much at risk of burnout as musicians or athletes. Parents, teachers, and the students themselves should be on the lookout for signs of decreased interest, and they must be willing to back off and allow the student to rediscover an interest in mathematics on his or her own. Burnout is particularly pernicious because th e end result often isn’t a backlash against competition, but against math in general. Indeed, even students not involved in contests have to watch out for burnout, though the pressure of contests tends to encourage burnout more quickly than the classroom.These possible perils are usually more than offset not only by the values we’ve already mentioned, but also by the greatest asset of math contests - cooperation. These competitions bring together students of like interests and abilities, allowing them to form their own community in which they will find friendship, inspiration, and encouragement to a far greater degree than most of these students can find in the typical classroom. Whereas a student may be one of only three or four in her school who pursues math the way others play basketball, she will not find herself so lonely at a math contest, where she’ll find many kindred spirits.In summary, math contests are a tremendous social and intellectual opportunity for students, but exposingstudents to contests must be done wisely, else they become counterproductive to the goal of encouraging a lifelong interest in mathematics and other intellectual pursuits.Direction (Note: For this question, please write your answer on fi le “high-school-answersheet.doc”, downloadable together with this document at , and submit file“high-school-answersheet.doc” at after you are done.):In his article “Pros and Cons of Math Competitions”, Richard Rusczyk lists and explains the advantages and the disadvantages of math competitions. Are math contests beneficial or harmful to students? Everything always has two sides: the good and the bad. This proverb probably also applies to math competitions. Write a response in which you discuss whether students should be encouraged to participate in math competitions. You may draw examples from your reading, studies, experience, observations, and etc.Hint: Here are some questions to think about. You do not have to answer them, but they will help you to craft your response.1. What is the purpose of math contests?2. What are the good and the bad sides of math contests?3. How should you value your scores on math contests?4. What are the most important qualities that a successful mathematician should have?5. To a student who is highly interested in mathematics, what are much more important than math contests?。
2014-2015美国大联盟五年级

2014-2015年度美国“数学大联盟杯赛”(中国赛区)初赛(五年级)中文版一、选择题(每小题5分,答对加5分,答错不扣分,175分,请将正确答案A/B/C或者D 写在每题后面的圆括号内)8. 80+(160+240) ÷4=40+80+(120÷____ ) ()A. 4B. 2C. 1D. 09. 下列式子中哪个式子的余数最大? ()A. 1111 ÷8B. 2222 ÷7C. 3333 ÷6D. 4444 ÷510. 下列各数中,哪个是20×14×20×15的因数? ()A. 13B. 11C. 9D. 711. Thok有一个简单的计划。
他准备花费一天中50%的时间在洞穴中,剩下的时间中的25%用来打猎,剩余的时间在外面看电影。
那么他将花费多少时间看电影呢? ()A. 3B. 6C. 9D. 2512. 2×3×6×36×2×3×6×36=()?A. 65B. 66C. 67D. 6813. 我有5个1美分的便士,4个5美分的硬币,3个0.25的硬币,2个0.5美元的硬币和1美元。
那这些硬币的平均值是多少()?A. 0.02美元B.0.06美元C. 1.5美元D. 3美元14. Wyatt O’Vine的羊的体重是Wyatt的两倍,Wyatt的体重是他帽子的两倍,如果Wyatt,羊,他的帽子体重在一起时210kg,那Wyatt重多少? ()A. 30kgB. 35kgC. 60kgD. 70kg15. (12+34)×(56+78)=12×(56+78)+_____×(56+78) ? ()A. 12B. 34C. 56D. 7816. 如果2个群等于5个斑点,那么500个群等于______个斑点。
()A. 200B. 250C. 1000D. 125017.(64+64)2 =()A. 16B. 64C. 128D. 25618. 如果7个连续的偶数和是182,那么7个数中最小的数字是()A. 20B. 23C. 26D. 3219. 当他倒立时,Flip决定从777开始每8个数字一倒数,那以下的哪个数字他会数到? ()A. 123B. 125C. 127D. 12920. 买5个苹果和买6个梨的价格是一样的,如果一个苹果比一个梨多花15美分,那么5个苹果和6个梨在一起一共多少钱? ()A. 3美元B. 6美元C. 9美元D. 18美元21. 27和27所有因数的乘积之间相差多少? ()A. 2B. 27C. 2×27D. 26×2722. 一个小于100的最大素数分解数最多是_____个素数的乘积(不一定是不同的)? ()A. 3B. 4C. 5D. 623. 一个四边都是整数边的长方形被分成了一个正方形和一块阴影的长方形。
2014-2015年度美国”数学大联盟杯赛“(中国赛区)初赛-(十、十一、十二年级).doc

2014-2015年度美国”数学大联盟杯赛“(中国赛区)初赛(十、十一、十二年级)一、选择题(每小题10分,答对加10分,答错不扣分,共100分,请将正确答案A 、B 、C 或者D 写在每题后面的圆括号内。
)正确答案填写示例如下:=-⨯⨯20522 ? (A )A)5 B)15 C)25 D)301. Meg loves her megaphone! The large circular end has a circumference that is the reciprocal of its diameter. What is the area of the circle? ( )A)π14 B) π12 C) 14 D) 122. How many solutions does the equation x x +=233 have? ( )A)0 B)1 C)2 D)43. If y x =-1, which of the following is always true for any value of x ? ( )A) ()()x y -=-2211B) ()()x x y y -=-222211 C) ()()x x y y --=-222211 D) ()()()()x x y y -+=-+22221111 4. Lee the crow ate a grams of feed that was 1% seed, b grams of feed that was 2% seed, and c grams of feed that was 3% seed. If combined, all the feed he ate was 1.5% seed. What is a in terms of b and c ?( )A)b c +3B)b c +3 C)b c +23 D)b c +32 5. If <x 0 and <.x 2001, then x -1 must be ( )A)less than -10B)between-0.1 and 0 C)between 0and 0.1 D) greater than 106. At 9:00 A.M., the ratio of red to black cars in a parking lot was 1 to 5. An hour later the number of red cars had increased by 2, the number of black cars had decreased by 5, and the ratio of red to black cars was 1 to 4. How many black cars were in the lot at 10:00 A.M.? ( )A)13 B)15 C)60 D)657. If x ≠1and x ≠-1, then ()()()x x x x x --++-32241111=( ) A)x -21 B) x +21 C) x -241 D) x -341 8. The Camps are driving at a constant rate. At noon they had driven 300 km.At 3:30 P.M. they had driven 50% further than they had driven by 1:30 P.M.What is their constant rate in km/hr? ( )A)150 B)120 C)100 D)909. The letters in DIGITS can be arranged in how many orders without adjacent I ’s? ( )A)240 B)355 C)600 D)71510. Al, Bea, and Cal each paint at constant rates, and together they are painting a house. Al and Bea togethercould do the job in 12 hours; Al and Cal could to it in 15, and Bea and Cal could do it in 20. How many hours will it take all three working together to paint the house?( )A)8.5 B)9 C)10 D)10.5二、填空题(每小题10分,答对加10分,答错不扣分,共200分)11. What is the sum of the degree-measures of the angles at the outer points ,,,A B C D and E of a five-pointed star, as shown? Answer: . 12. What is the ordered pair of positive integers (,k b ), with the least value of k , which satisfiesk b ⋅⋅=34234?Answer: .13. A face-down stack of 8 playing cards consisted of 4 Aces (A ’s) and 4 Kings (K ’s).After I revealed and then removed the top card, I moved the new top card to thebottom of the stack without revealing the card. I repeated this procedure until thestack without revealing the card. I repeated this procedure until the stack was leftwith only 1 card, which I then revealed. The cards revealed were AKAKAKAK ,in that order. If my original stack of 8 cards had simply been revealed one card at atime, from top to bottom (without ever moving cards to the bottom of the stack),in what order would they have been revealed?Answer: .14. For what value of a is one root of ()x a x a -+++=222120 twice the other root?Answer: .15. Each time I withdrew $32 from my magical bank account, the account ’sremaining balance doubled. No other account activity was permitted. My fifth$32 withdrawal caused my account ’s balance to become $0. With how manydollars did I open that account?Answer: .16. In how many ways can I select six of the first 20 positive integers, disregarding the order in which these sixintegers are selected, so that no two of the selected integers are consecutive integers?Answer: .17. If, for all real ,()()xx f x f x =-21, what is the numerical value of f (3)?Answer: .18. How many pairs of positive integers (without regard to order) have a least common multiple of 540?Answer: .19. If the square of the smaller of consecutive positive integers is x , what is the square of the larger of thesetwo integers, in terms of x ?Answer: .20. A pair of salt and pepper shakers comes in two types: identical and fraternal.Identical pairs are always the same color. Fraternal pairs are the same colorhalf the time. The probability that a pair of shakers is fraternal is p andthat a pair is identical is .q p =-1 If a pair of shakers is of the same color, AE DCBword 格式-可编辑-感谢下载支持 determine, in terms of the variable q alone, the probability that the pair is identical. Answer: .21. As shown, one angle of a triangle is divided into four smaller congruentangles. If the lengths of the sides of this triangle are 84, 98, and 112, as shown,how long is the segment marked x ?Answer: .22. How long is the longer diagonal of a rhombus whose perimeter is 60, if threeof its vertices lie on a circle whose diameter is 25, as shown?Answer: .23. The 14 cabins of the Titanic Mail Boat are numbered consecutively from1 through 14, as are the 14 room keys. In how many different ways canthe 14 room keys be placed in the 14 rooms, 1 per room, so that, for everyroom, the sum of that room ’s number and the number of the key placed inthat room is a multiple of 3?Answer: .24. For some constant b , if the minimum value of ()x x b f x x x b -+=++2222is 12, what is the maximum value of ()f x ? Answer: .25. If the lengths of two sides of a triangle are 60cos A and 25sin A , what is the greatest possible integer-length of the third side?Answer: .26. {}n a is a geometric sequence in which each term is a positive number. If a a =5627, what is the value oflog log log ?a a a +++3132310Answer: . 27. What is the greatest possible value of ()=sin cos ?f x x x ++3412Answer: .28. Let C be a cube. Triangle T is formed by connecting the midpoints of three edges of cube C . What is the greatest possible measure of an angle of triangle T ?Answer: .29. Let a and b be two real numbers. ()sin f x a x b x =++34 and (lg log )f =3105. What is the value of (lg lg )f 3?Answer: .30. Mike likes to gamble. He always bets all his chips whenever the number of chips he has is <=5. He always bets n (10-)chips whenever the number of chips he has is greater than 5 and less than 10. He continues betting until either he has no chips or he has more than 9 chips. For every round, if he bets n chips. The probability that he wins or loses in each round is 50%. If Mike begins with 4 chips, what is the probability that he loses all his chips?Answer: .1129884xword格式-可编辑-感谢下载支持。
2015 AMC 12A 考题及答案

2015AMC12A考题及答案Problem1What is the value ofProblem2Two of the three sides of a triangle are20and15.Which of the following numbers is not a possible perimeter of the triangle?Problem3Mr.Patrick teaches math to15students.He was grading tests and found that when he graded everyone's test except Payton's,the average grade for the class was80.After he graded Payton's test,the class average became 81.What was Payton's score on the test?Problem4The sum of two positive numbers is5times their difference.What is the ratio of the larger number to the smaller?Problem5Amelia needs to estimate the quantity,where and arelarge positive integers.She rounds each of the integers so that the calculation will be easier to do mentally.In which of these situations will her answer necessarily be greater than the exact value of?Problem6Two years ago Pete was three times as old as his cousin Claire.Two years before that,Pete was four times as old as Claire.In how many years will the ratio of their ages be?Problem7Two right circular cylinders have the same volume.The radius of the second cylinder is more than the radius of the first.What is therelationship between the heights of the two cylinders?Problem8The ratio of the length to the width of a rectangle is:.If therectangle has diagonal of length,then the area may be expressed asfor some constant.What is?Problem9A box contains2red marbles,2green marbles,and2yellow marbles. Carol takes2marbles from the box at random;then Claudia takes2of the remaining marbles at random;and then Cheryl takes the last2marbles. What is the probability that Cheryl gets2marbles of the same color?Problem10Integers and with satisfy.What is?Problem11On a sheet of paper,Isabella draws a circle of radius,a circle of radius ,and all possible lines simultaneously tangent to both circles.Isabella notices that she has drawn exactly lines.How many differentvalues of are possible?Problem12The parabolas and intersect the coordinate axes in exactly four points,and these four points are the vertices of a kite of area.What is?Problem13A league with12teams holds a round-robin tournament,with each team playing every other team exactly once.Games either end with one team victorious or else end in a draw.A team scores2points for every game it wins and1point for every game it draws.Which of the following is NOT a true statement about the list of12scores?Problem14What is the value of for which?Problem15What is the minimum number of digits to the right of the decimal pointneeded to express the fraction as a decimal?Problem16Tetrahedron hasand.What is the volume of the tetrahedron?Problem17Eight people are sitting around a circular table,each holding a fair coin. All eight people flip their coins and those who flip heads stand while those who flip tails remain seated.What is the probability that no two adjacent people will stand?Problem18The zeros of the function are integers.What is the sum of the possible values of?Problem19For some positive integers,there is a quadrilateral withpositive integer side lengths,perimeter,right angles at and,,and.How many different values of arepossible?Problem20Isosceles triangles and are not congruent but have the same areaand the same perimeter.The sides of have lengths of and,while those of have lengths of and.Which of the followingnumbers is closest to?Problem21A circle of radius passes through both foci of,and exactly four pointson,the ellipse with equation.The set of all possible values of is an interval.What is?Problem22For each positive integer,let be the number of sequences of length consisting solely of the letters and,with no more thanthree s in a row and no more than three s in a row.What is theremainder when is divided by12?Problem23Let be a square of side length1.Two points are chosen independentlyat random on the sides of.The probability that the straight-linedistance between the points is at least is,where andare positive integers and.What is?Problem24Rational numbers and are chosen at random among all rationalnumbers in the interval that can be written as fractions whereand are integers with.What is the probabilitythat is a real number?Problem25A collection of circles in the upper half-plane,all tangent to the-axis,is constructed in layers as yer consists of two circles of radiiand that are externally tangent.For,the circles inare ordered according to their points of tangency with the-axis.For every pair of consecutive circles in this order,a new circle is constructed externally tangent to each of the two circles in the yerconsists of the circles constructed in this way.Let,andfor every circle denote by its radius.What is2015AMC12A Answer Key1.C2.E3.E4.B5.D6.B7.D8.C9.C10.E11.D12.B13.E14.D15.C16.C17.A18.C19.B20.A21.D22.D23.A24.D25.D。
2015AMC10A试题及详解(繁体中文版)

14 16 8 3 E 8 16
2
故符合題意之樣本 p 16 q 16 r n 4 10 1 10
p 0 , 1 , 2 , 3 q 0~9 r 0~9
399 種
p q r 0
故 n 的所有位數(以 10 為底)的數字和 3 9 9 21
3. 小安用 18 根牙籤排出一個 3 階的樓梯,如圖所示,試問小安還要 多加幾根牙籤才能排出一個 5 階的樓梯? (A) 9 (B) 18 (C) 20 (D) 22 (E) 24 。 【2015AMC10A】
答: (D) 解: a 1 4 , a 2 10 , a 3 18 , a 4 28 , a 5 40
答: (D)
x 解: CA CB x 12.5 x 5 2 25 1 1 ABC 5 CD sin 30 5 CD sin 60 CD 2 2 2 CDE 1 2 3 1 10
2
10 3 1
2
11. 某個長方形長與寬的比為 4 : 3 ,若此長方形對角線的長為 d ,且其面積等於 kd , 則 k 之值為何? 2 3 12 16 3 (A) (B) (C) (D) (E) 。 【2015AMC10A】 7 7 25 25 4 答: (C) 解: 長 4 t ,寬 3 t ,對角線 d 5 t
1 1 5 25 5
2. 盒中裝有三角形與正方形的紙片,若盒中總共有 25 張紙片,且這些紙片總共有 84 條邊, 則盒中有多少張正方形紙片? (A) 3 (B) 5 (C) 7 (D) 9 (E) 11 。 【2015AMC10A】
答: (D) x 三角形 16 x y 25 解: 3 x 4 y 84 y 正方形 9
AMC 10 2015A真题

1.What is the value of2. A box contains a collection of triangular and square tiles. There are tiles in the box, containingedges total. How many square tiles are there in the box?3.Ann made a 3-step staircase using 18 toothpicks as shown in the figure. How many toothpicks does sheneed to add to complete a 5-step staircase?4.Pablo, Sofia, and Mia got some candy eggs at a party. Pablo had three times as many eggs as Sofia, andSofia had twice as many eggs as Mia. Pablo decides to give some of his eggs to Sofia and Mia so that all three will have the same number of eggs. What fraction of his eggs should Pablo give to Sofia?5.Mr. Patrick teaches math to students. He was grading tests and found that when he graded everyone'stest except Payton's, the average grade for the class was . After he graded Payton's test, the test average became . What was Payton's score on the test?6.The sum of two positive numbers is times their difference. What is the ratio of the larger number to thesmaller number?7.How many terms are there in the arithmetic sequence , , , . . ., , ?8.Two years ago Pete was three times as old as his cousin Claire. Two years before that, Pete was fourtimes as old as Claire. In how many years will the ratio of their ages be : ?9.Two right circular cylinders have the same volume. The radius of the second cylinder is more thanthe radius of the first. What is the relationship between the heights of the two cylinders?10.How many rearrangements of are there in which no two adjacent letters are also adjacent letters inthe alphabet? For example, no such rearrangements could include either or .11.The ratio of the length to the width of a rectangle is : . If the rectangle has diagonal of length ,then the area may be expressed as for some constant . What is ?12.Points and are distinct points on the graph of . What is?13.Claudia has 12 coins, each of which is a 5-cent coin or a 10-cent coin. There are exactly 17 differentvalues that can be obtained as combinations of one or more of her coins. How many 10-cent coins does Claudia have?14.The diagram below shows the circular face of a clock with radius cm and a circular disk with radiuscm externally tangent to the clock face at o'clock. The disk has an arrow painted on it, initially pointing in the upward vertical direction. Let the disk roll clockwise around the clock face. At what point on the clock face will the disk be tangent when the arrow is next pointing in the upward verticaldirection?15.Consider the set of all fractions where and are relatively prime positive integers. How many ofthese fractions have the property that if both numerator and denominator are increased by , the value of the fraction is increased by ?16.If , and , what is the value of ?17.A line that passes through the origin intersects both the line and the line . Thethree lines create an equilateral triangle. What is the perimeter of the triangle?18.Hexadecimal (base-16) numbers are written using numeric digits through as well as the lettersthrough to represent through . Among the first positive integers, there are whose hexadecimal representation contains only numeric digits. What is the sum of the digits of ?19.The isosceles right triangle has right angle at and area . The rays trisectingintersect at and . What is the area of ?20.A rectangle with positive integer side lengths in has area and perimeter . Which of thefollowing numbers cannot equal ?NOTE: As it originally appeared in the AMC 10, this problem was stated incorrectly and had no answer; it has been modified here to be solvable.21.Tetrahedron has , , , , , and .What is the volume of the tetrahedron?22.Eight people are sitting around a circular table, each holding a fair coin. All eight people flip their coinsand those who flip heads stand while those who flip tails remain seated. What is the probability that no two adjacent people will stand?23.The zeroes of the function are integers. What is the sum of the possible valuesof ?24.For some positive integers , there is a quadrilateral with positive integer side lengths,perimeter , right angles at and , , and . How many different values of are possible?25.Let be a square of side length . Two points are chosen at random on the sides of . The probabilitythat the straight-line distance between the points is at least is , where , , and are positive integers with . What is ?。
