ABAQUS地震反应谱分析

合集下载

ABAQUS钢框架结构抗震仿真分析

ABAQUS钢框架结构抗震仿真分析D才建好的ac加速度，OK。

7、MeshSeed Part Instance；Assign Mesh Controls：Quard，Free，Advancing front；Assign Element Type：Standard，Linear，Reduced Integration，壳单元S4R；Mesh Part；最后Verify Mesh检查网格是否错误。

8、JobCreate Job；Write Input（输出inp文件）,Submit；打开Monitor观察是否有错误、警报；点击Result进入可视化窗口Visualization。

9、VisualizationResult，Step/Frame分析步/帧，查看各时程变形情况；Tools，XY Data，Create,ODB field output，plot顶层楼板位移时程曲线，各层楼板加速度时程曲线，各层楼板位移曲线。

10、数据后处理在Visualization，XY Data Manager中点击Edit，输出各层楼板位移数据到Excel中，利用Excel：相邻两层的位移作差后得到层间相对位移，画出以Time为横轴、层间相对位移为纵轴画出各层层间位移时程曲线；然后层间相对位移除以层高得到层间位移角，画出以Time为横轴、层间位移角为纵轴画出各层层间位移角时程曲线。

四、结果展示钢框架内力云图1钢框架内力云图2钢框架内力云图3顶层楼板位移时程曲线底端加速度时程曲线一层楼板加速度时程曲线二层楼板加速度时程曲线三层楼板加速度时程曲线四层楼板加速度时程曲线五层楼板加速度时程曲线顶层楼板加速度时程曲线底端与一层楼板层间位移一层与二层楼板层间位移二层与三层楼板层间位移三层与四层楼板层间位移四层与五层楼板层间位移五层与顶层楼板层间位移底端与一层楼板层间位移角一层与二层楼板层间位移角二层与三层楼板层间位移角三层与四层楼板层间位移角四层与五层楼板层间位移角五层与六层楼板层间位移角五、总结与分析通过分析数据：①该钢框架模型顶层楼板最大位移发生在9.904615s时，值为0.521279；根据《钢结构设计规范》:多层框架结构柱顶位移H/500，对于该模型为18m/500=0.036m，顶层楼板最大位移超出规范要求。

地震反应分析

结构地震反应分析结构地震反应分析的主要工作是首先将结构简化成力学分析模型，然后输入地震作用，计算模拟结构的反应行为，包括内力和变形反应时程或最大值。

其目的是为结构抗震设计提供必要的数据资料；或为抗震安全鉴定和拟定抗震加固方案提供参考依据；或为研究结构破坏机理提供基本手段，从而改善设计，提高结构的抗震性能。

结构地震反应取决于地震动输入特性和结构特性。

随着人们对地震动特性和结构特性的了解越来越多，特别是技术手段越来越先进，结构地震反应分析方法也跟着有了飞跃的发展。

结构抗震分析方法的发展大体上可分为三个阶段，即静力法、拟静力法（通常指反应谱方法）和动力法阶段。

静力法是20世纪初首先在日本发展起来的。

该方法将结构物看成是刚体，并刚接于地面。

这样，结构在最大水平加速度绝对值为max a 的地面运动激励下，受到的最大水平作用力P （即最大惯性力）为kW A gW P ==max 其中，W 是结构物的重量，k 是地面最大水平加速度绝对值max A 与重力加速度g 之比，称为地震系数。

在当时人们对地面运动的频谱和卓越周期的了解还不够多，以及房屋多为低层建筑的情况下，应用上述地震荷载计算公式于抗震设计还是可以的。

但是，随着地震资料的积累和城市与工业建设的发展，使人们认识到作为静力法基础的刚性结构假定已明显地远离实际情况，于是考虑结构物的弹性性质、阻尼性质及相应动力特性的反应谱方法便发展起来了。

反应谱方法出现在20世纪40年代。

美国的一些学者在取得了一部分强震地面运动记录之后，考虑地震动特性与结构动力特性共同对结构地震反应产生决定性影响的这一事实，提出了反应谱概念和相应的设计计算方法。

这一方法有动力法的内容，却具静力法的形式，故可称之为拟静力法。

该方法对结构地震反应分析产生巨大影响，至今仍是结构抗震设计的主要计算方法。

尽管反应谱方法取得的进步是实质性的，但它的应用还是受到一些限制，如原则上只能用于线性结构体系；不能真实反映复杂结构体系的动力放大作用。

ABAQUS时程分析法计算地震反应的简单实例

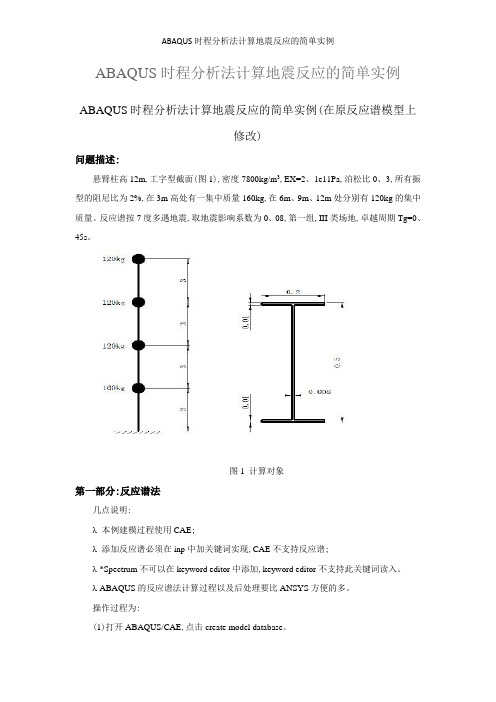
ABAQUS时程分析法计算地震反应的简单实例ABAQUS时程分析法计算地震反应的简单实例(在原反应谱模型上修改)问题描述:悬臂柱高12m,工字型截面(图1),密度7800kg/m3,EX=2、1e11Pa,泊松比0、3,所有振型的阻尼比为2%,在3m高处有一集中质量160kg,在6m、9m、12m处分别有120kg的集中质量。

反应谱按7度多遇地震,取地震影响系数为0、08,第一组,III类场地,卓越周期Tg=0、45s。

图1 计算对象第一部分:反应谱法几点说明:λ本例建模过程使用CAE;λ添加反应谱必须在inp中加关键词实现,CAE不支持反应谱;λ *Spectrum不可以在keyword editor中添加,keyword editor不支持此关键词读入。

λ ABAQUS的反应谱法计算过程以及后处理要比ANSYS方便的多。

操作过程为:(1)打开ABAQUS/CAE,点击create model database。

(2)进入Part模块,点击create part,命名为column,3D、deformation、wire。

continue(3) Create lines,在分别输入0,0回车;0,3回车;0,6回车;0,9回车;0,12回车。

(4)进入property模块,create material,name:steel,general-->>density,mass density:7800mechanical-->>elasticity-->>elastic,young‘s modulus:2、1e11,poisson’s ratio:0、3、(5) Create section,name:Section-1,category:beam,type:beam,Continuecreate profile, name:Profile-1, shape:I,按图1尺寸输入界面尺寸,ok。

abaqus 地下结构抗震反应位移法

Abaqus 地下结构抗震反应位移法一、引言地下结构的抗震设计一直是工程领域的热门话题，地下结构在地震作用下可能受到严重破坏，因此需要对其进行抗震设计和分析。

而其中的反应位移法在地下结构的抗震分析中得到了广泛的应用，Abaqus 软件作为一款强大的有限元分析工具，在地下结构抗震反应位移法中也具有很高的应用价值。

本文将对Abaqus软件在地下结构抗震反应位移法中的应用进行系统的介绍。

二、地下结构抗震分析的重要性1. 地下结构在工程领域中的重要性地下结构作为现代城市建设的重要组成部分，在城市的供水、供热、排水、交通、防护等方面都发挥着重要作用。

而地下结构在地震作用下的破坏可能会给城市的安全和稳定带来严重影响，因此对地下结构进行抗震分析和设计具有重要意义。

2. 抗震分析的必要性地震是一种常见的自然灾害，具有突发性和破坏性。

地震作用下地下结构可能受到严重的破坏，因此需要进行抗震分析和设计来保证地下结构在地震作用下的安全性。

三、Abaqus软件在地下结构抗震反应位移法中的应用1. 地下结构抗震分析的基本原理地下结构抗震分析主要是研究地下结构在地震作用下的受力和变形情况，通过分析地下结构的地震响应，评估地下结构的抗震性能。

在地下结构抗震分析中，反应位移法是一种常用的分析方法，它是通过建立地下结构的受力平衡方程和动力平衡方程，利用结构的刚度矩阵和地震激励谱，计算地下结构在地震作用下的位移响应。

2. Abaqus软件在地下结构抗震分析中的优势Abaqus软件作为一款强大的有限元分析工具，具有很高的分析精度和计算效率，在地下结构抗震分析中具有很强的应用价值。

Abaqus 软件可以实现地下结构的三维动力分析，在考虑地震激励的情况下，计算地下结构在地震作用下的动力响应。

3. Abaqus软件在地下结构抗震反应位移法中的具体应用Abaqus软件在地下结构抗震反应位移法中具体包括以下几个方面的应用：（1）建立地下结构的有限元模型。

ABAQUS时程分析法计算地震反应的简单实例

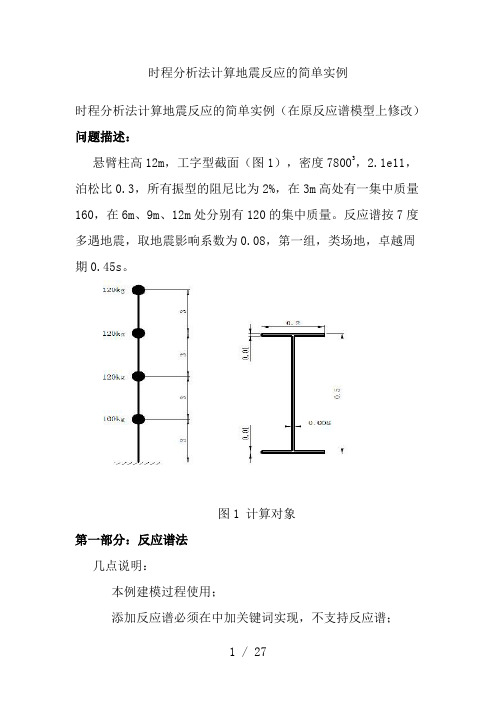
时程分析法计算地震反应的简单实例时程分析法计算地震反应的简单实例（在原反应谱模型上修改）问题描述：悬臂柱高12m，工字型截面（图1），密度78003，2.1e11，泊松比0.3，所有振型的阻尼比为2%，在3m高处有一集中质量160，在6m、9m、12m处分别有120的集中质量。

反应谱按7度多遇地震，取地震影响系数为0.08，第一组，类场地，卓越周期0.45s。

图1 计算对象第一部分：反应谱法几点说明：本例建模过程使用；添加反应谱必须在中加关键词实现，不支持反应谱；*不可以在中添加，不支持此关键词读入。

的反应谱法计算过程以及后处理要比方便的多。

操作过程为：（1）打开，点击。

（2）进入模块，点击，命名为，3D、、。

（3），在分别输入0，0回车；0，3回车；0，6回车；0，9回车；0，12回车。

（4）进入模块，，：，>>，：7800>>>>，‘s ：2.1e11，’s ：0.3.（5），：1，：，：,, : 1, ,按图1尺寸输入界面尺寸，。

在选择I，选择。

（6），选择全部，，弹出的对话框选择：1，。

（7），选择全部，默认值确定。

（8） >> ，在弹出的对话框里勾选，，以可视化梁截面形状。

（9）添加集中质量，>>>>，：1，：，，选择（0，3）位置点，：160，。

，：2，：，，选择0，6；0，9；0，12位置点（按多选），，：120，，。

（10） >> ，选（），。

（11） >> ，：1，选，在选项卡中，选择频率提取方法，本例选用法，，选，输入10。

再，，：2，选，在选项卡中，选择单向，选择（）法，：(反应谱的，后面再中添加)，方向余弦（0，0，1），：1.进入选项卡，阻尼使用直接模态（），勾选，：1，：8，：0.02。

（12）进入模块，>> ，：，选择，选择，选择，选择0，0点，，勾选u13所有6个自由度。

ABAQUS钢框架结构抗震仿真分析

ABAQUS钢框架结构抗震仿真分析首先，我们需要建立结构的有限元模型。

钢框架结构主要由柱、梁、节点和连接件组成，我们需要根据实际情况进行建模。

在ABAQUS中，我们可以使用节点（节点）和单元（单元）建立结构模型。

其次，我们需要定义结构的材料特性。

在钢框架结构中，材料的弹性模量（E）和泊松比（ν）是两个重要参数。

根据实际材料的特性，我们可以在ABAQUS中定义这些参数。

接下来，我们需要定义结构的边界条件。

抗震仿真分析通常需要在地震力作用下进行，我们需要定义结构的固定支撑条件，以模拟垂直方向上的地震力。

在ABAQUS中，我们可以将结构的底部或其他特定地方固定支撑。

然后，我们需要定义地震载荷。

地震力通常由地震加速度谱表示，在ABAQUS中，我们可以通过载荷定义来输入这些数据。

根据地震保护设计准则，我们可以计算出地震力对结构的作用。

在进行抗震仿真分析之前，我们还需要进行网格划分和网格优化。

钢框架结构通常具有较高的刚度和复杂的形状，我们需要根据结构的实际情况进行网格划分，并使用ABAQUS的网格优化工具来确保网格质量。

最后，我们可以进行抗震仿真分析。

在此过程中，我们可以将地震载荷应用于结构，并模拟结构在地震力作用下的响应。

ABAQUS可以计算出结构的位移、应力和变形等参数，并可生成相应的结果报告。

总结起来，ABAQUS是一种强大的有限元分析工具，可以用于钢框架结构的抗震仿真分析。

通过建立模型、定义材料特性、边界条件和地震载荷，进行网格划分和网格优化，并进行仿真分析，我们可以获取结构在地震力作用下的响应情况，评估结构的抗震性能，并指导实际工程设计。

ABAQUS软件在基于性能的地震时程分析上的应用

ABAQUS软件在基于性能的地震时程分析上的应用一、本文概述随着科技的发展和工程需求的提升，基于性能的地震时程分析（Performance-Based Earthquake Engineering, PBEE）已成为结构工程领域的研究热点。

在这种分析方法中，结构的抗震性能不再仅通过传统的承载能力来评估，而是更多地关注结构在地震作用下的实际表现，如变形、耗能等。

这为结构设计和抗震评估提供了新的视角和更高的要求。

ABAQUS软件作为一款功能强大的有限元分析软件，能够模拟结构在各种复杂工况下的力学行为，因此在基于性能的地震时程分析中得到了广泛应用。

本文旨在探讨ABAQUS软件在PBEE中的应用，介绍其基本原理、分析流程、关键技术及实际案例。

通过对ABAQUS软件在地震时程分析中的具体实践进行详细阐述，期望能为工程师和研究人员提供有益的参考和启示，推动基于性能的地震工程研究的深入发展。

二、ABAQUS软件简介ABAQUS是一款功能强大的工程模拟软件，广泛应用于各种工程领域的复杂问题求解，包括结构力学、流体动力学、热力学、电磁学等。

其强大的分析能力主要源于其丰富的材料模型库、精确的求解器以及灵活的用户界面。

ABAQUS以其高度的精确性和可靠性，在科研和工程实践中得到了广泛应用。

在结构工程领域，ABAQUS提供了丰富的单元类型和材料模型，可以满足从简单线性问题到复杂非线性问题的模拟需求。

其中，ABAQUS/Standard模块用于处理一般的线性和非线性问题，而ABAQUS/Explicit模块则特别适用于处理涉及冲击、爆炸等高度非线性动力学问题。

ABAQUS还提供了丰富的接触和连接类型，可以模拟各种复杂的结构连接形式。

在地震工程领域，ABAQUS的动力学分析能力尤为突出。

它不仅可以进行模态分析、反应谱分析等线性动力学分析，还可以进行直接积分法等非线性动力学分析。

这使得ABAQUS能够准确模拟地震波在结构中的传播过程，以及结构在地震作用下的动力响应。

abaqus中地动态分析报告方法

ABAQUS线性动态分析如果你只对结构承受载荷后的长期响应感兴趣，静力分析（static analysis）是足够的。

然而，如果加载时间很短（例如在地震中）或者如果载荷在性质上是动态的（例如来自旋转机械的荷载），你就必须采用动态分析（dynamic analysis）。

本章将讨论应用ABAQUS/Standard进行线性动态分析；关于应用ABAQUS/Explicit进行非线性动态分析的讨论，请参阅第9章“非线性显式动态分析”。

7.1 引言动态模拟是将惯性力包含在动力学平衡方程中：其中M结构的质量。

u结构的加速度。

I在结构中的内力。

P 所施加的外力。

在上面公式中的表述是牛顿第二运动定律（F = ma）。

在静态和动态分析之间最主要的区别是在平衡方程中包含了惯性力（M u）。

在两类模拟之间的另一个区别在于内力I的定义。

在静态分析中，内力仅由结构的变形引起；而在动态分析中，内力包括源于运动（例如阻尼）和结构的变形的贡献。

7.1.1 固有频率和模态最简单的动态问题是在弹簧上的质量自由振动，如图7-1所示。

图7–1 质量－弹簧系统在弹簧中的内力给出为ku ，所以它的动态运动方程为这个质量－弹簧系统的固有频率（natral frequency ）（单位是弧度/秒（rad/s ））给出为ω=如果质量块被移动后再释放，它将以这个频率振动。

若以此频率施加一个动态外力，位移的幅度将剧烈增加，这种现象即所谓的共振。

实际结构具有大量的固有频率。

因此在设计结构时，非常重要的是避免使可能的载荷频率过分接近于固有频率。

通过考虑非加载结构（在动平衡方程中令0P =）的动态响应可以确定固有频率。

则运动方程变为对于无阻尼系统，I Ku =，因此有这个方程的解具有形式为t i e u ωφ=将此式代入运动方程，得到了特征值（eigenvalue ）问题其中2λω=。

该系统具有n 个特征值，其中n 是在有限元模型中的自由度数目。

记j λ是第j 个特征值；它的平方根j ω是结构的第j 阶模态的固有频率（natural frequency ），而j φ是相应的第j 阶特征向量（eigenvector ）。

abaqus帮助文档之地震相应计算分析

2.1.15Seismic analysis of a concrete gravity damProducts:Abaqus/Standard Abaqus/ExplicitIn this example we consider an analysis of the Koyna dam, which was subjected to an earthquake of magnitude 6.5 on the Richter scale on December 11, 1967. The example illustrates a typical application of the concrete damaged plasticity material model for the assessment of the structural stability and damage of concrete structures subjected to arbitrary loading. This problem is chosen because it has been extensively analyzed by a number of investigators, including Chopra and Chakrabarti(1973), Bhattacharjee andLéger(1993), Ghrib and Tinawi(1995), Cervera et al.(1996), and Lee and Fenves(1998). Problem descriptionThe geometry of a typical non-overflow monolith of the Koyna dam is illustrated in Figure 2.1.15–1. The monolith is 103m high and 71m wide at its base. The upstream wall of the monolith is assumed to be straight and vertical, which is slightly different from the real configuration. The depth of the reservoir at the time of the earthquake is= 91.75m. Following the work of other investigators, we consider a two-dimensional analysis of the non-overflow monolith assuming plane stress conditions. The finite element mesh used for the analysis is shown in Figure 2.1.15–2. It consists of 760 first-order, reduced-integration, plane stress elements (CPS4R). Nodal definitions are referred to a global rectangular coordinate system centered at the lower left corner of the dam, with the vertical y-axis pointing in the upward direction and the horizontal x-axis pointing in the downstream direction. The transverse and vertical components of the ground accelerations recorded during the Koyna earthquake are shown in Figure 2.1.15–3(units of g= 9.81m sec–2). Prior to the earthquake excitation, the dam is subjected to gravity loading due to its self-weight and to the hydrostatic pressure of the reservoir on the upstream wall. For the purpose of this example we neglect the dam–foundation interactions by assuming that the foundation is rigid. The dam–reservoir dynamic interactions resulting from the transverse component of ground motion can be modeled in a simple form using the Westergaard added mass technique. According to Westergaard (1933), the hydrodynamic pressures that the water exerts on the dam during an earthquake are the same as if a certain body of water moves back and forth with the dam while the remainder of the reservoir is left inactive. The added mass per unit area of theupstream wall is given in approximate form by the expression, with, where= 1000 kg/m3is the density of water. In the Abaqus/Standard analysis the added mass approach is implemented using a simple 2-node user element that has been coded in user subroutine UEL. In the Abaqus/Explicit analysis the dynamic interactions between the dam and the reservoir are ignored.The hydrodynamic pressures resulting from the vertical component of ground motion are assumed to be small and are neglected in all the simulations.Material propertiesThe mechanical behavior of the concrete material is modeled using the concrete damaged plasticity constitutive model described in“Concrete damaged plasticity,”Section 23.6.3 of the Abaqus Analysis User's Manual, and“Damaged plasticity model for concrete and other quasi-brittle materials,”Section 4.5.2 of the Abaqus Theory Manual. The material properties used for the simulations are given in Table 2.1.15–1and Figure 2.1.15–4. These properties are assumed to be representative of the concrete material in the Koyna dam and are based on the properties used by previous investigators. In obtaining some of these material properties, a number of assumptions are made. Of particular interest is the calibration of the concrete tensile behavior. The tensile strength is estimated to be 10% of the ultimate compressive strength (= 24.1MPa), multiplied by a dynamic amplification factor of 1.2 to account for rate effects; thus,= 2.9MPa. To avoid unreasonable mesh-sensitive results due to the lack of reinforcement in the structure, the tensile postfailure behavior is given in terms of a fracture energy cracking criterion by specifying a stress/displacement curve instead of a stress-strain curve, as shown in Figure 2.1.15–4(a). This is accomplished with the postcracking stress/displacement curve. Similarly, tensile damage,, is specified in tabular form as a function of cracking displacement by using the postcracking damage displacement curve. This curve is shown in Figure 2.1.15–4(b). The stiffness degradation damage caused by compressive failure (crushing) of the concrete,, is assumed to be zero.DampingIt is generally accepted that dams have damping ratios of about 2–5%. In this example we tune the material damping properties to provide approximately 3% fraction of critical damping for the first mode of vibration of the dam. Assuming Rayleigh stiffness proportional damping, the factor required to provide a fraction of critical damping for the first mode is givenas. From a natural frequency extraction analysis of the dam the first eigenfrequency is found to be= 18.61 rad sec–1(see Table 2.1.15–2). Based on this,is chosen to be 3.23 × 10–3sec.Loading and solution controlLoading conditions and solution controls are discussed for each analysis.Abaqus/Standard analysisPrior to the dynamic simulation of the earthquake, the dam is subjected to gravity loading and hydrostatic pressure. In the Abaqus/Standard analysis these loads are specified in two consecutive static steps, using a distributed load with the load type labels GRA V(for the gravity load) in the first step and HP(for the hydrostatic pressure) in the second step. For the dynamic analysis in the third step the transverse and vertical components of the ground accelerations shown in Figure 2.1.15–3are applied to all nodes at the base of the dam.Since considerable nonlinearity is expected in the response, including the possibility of unstable regimes as the concrete cracks, the overall convergence of the solution in the Abaqus/Standard analysis is expected to be non-monotonic. In such cases automatically setting the time incrementation parameters is generally recommended to prevent premature termination of the equilibrium iteration process because the solution may appear to be diverging. The unsymmetric matrix storage and solution scheme is activated by specifying an unsymmetric equation solver for the step. This is essential for obtaining an acceptable rate of convergence with the concrete damaged plasticity model since plastic flow is nonassociated. Automatic time incrementation isused for the dynamic analysis of the earthquake, with the half-increment residual tolerance set to 107and a maximum time increment of 0.02 sec.Abaqus/Explicit analysisWhile it is possible to perform the analysis of the pre-seismic state in Abaqus/Explicit, Abaqus/Standard is much more efficient at solving quasi-static analyses. Therefore, we apply the gravity and hydrostatic loads in an Abaqus/Standard analysis. These results are then imported into Abaqus/Explicit to continue with the seismic analysis of the dam subjected to the earthquake accelerogram. We still need to continue to apply the gravity and hydrostatic pressure loads during the explicit dynamic step. In Abaqus/Explicit gravity loading is specified in exactly the same way as in Abaqus/Standard. The specification of the hydrostatic pressure, however, requires some extra consideration because this load type is not currently supported by Abaqus/Explicit. Here we apply the hydrostatic pressure using user subroutine VDLOAD.The Abaqus/Explicit simulation requires a very large number of increments since the stable time increment (6 × 10–6sec) is much smaller than the total duration of the earthquake (10 sec). The analysis is run in double precision to prevent the accumulation of round-off errors. The stability limit could be increased by using mass scaling; however, this may affect the dynamic response of the structure.For this particular problem Abaqus/Standard is computationally more effective than Abaqus/Explicit because the earthquake is a relatively long event that requires a very large number of increments in Abaqus/Explicit. In addition, the size of the finite element model is small, and the cost of each solution of the global equilibrium equations in Abaqus/Standard is quite inexpensive.Results and discussionThe results for each analysis are discussed in the following sections.Abaqus/Standard resultsThe results from a frequency extraction analysis of the dam without the reservoir are summarized in Table 2.1.15–2. The first four natural frequencies of the finite element model are in good agreement with the values reported by Chopra and Chakrabarti(1973). As discussed above, the frequency extraction analysis is useful for the calibration of the material damping to be used during the dynamic simulation of the earthquake.Figure 2.1.15–5shows the horizontal displacement at the left corner of the crest of the dam relative to the ground motion. In this figure positive values represent displacement in the downstream direction. The crest displacement remains less than 30mm during the first 4 seconds of the earthquake. After 4seconds, the amplitude of the oscillations of the crest increases substantially. As discussed below, severe damage to the structure develops during these oscillations.The concrete material remains elastic with no damage at the end of the second step, after the dam has been subjected to the gravity and hydrostatic pressure loads. Damage to the dam initiates during the seismic analysis in the third step. The evolution of damage in the concrete dam at six different times during the earthquake is illustrated in Figure 2.1.15–6,Figure 2.1.15–7, and Figure 2.1.15–8. Times= 3.96sec,= 4.315sec, and= 4.687sec correspond to the first three large excursions of the crest in the upstream direction, as shown in Figure 2.1.15–5. Times= 4.163sec and= 4.526sec correspond to the first two large excursions of the crest in the downstream direction. Time= 10 sec corresponds to the end of the earthquake. The figuresshow the contour plots of the tensile damage variable,DAMAGET(or), on the left, and the stiffness degradation variable,SDEG(or d), on the right. The tensile damage variable is a nondecreasing quantity associated with tensile failure of the material. On the other hand, the stiffness degradation variable can increase or decrease, reflecting the stiffness recovery effects associated with the opening/closing of cracks. Thus, assuming that there is no compressive damage (), the combination and at a given material point represents an open crack, whereas and represents a closed crack.At time, damage has initiated at two locations: at the base of the dam on the upstream face and in the region near the stress concentration where the slope on the downstream face changes. When the dam displaces toward the downstream direction at time, the damage at the base leads to the formation of a localized crack-like band of damaged elements. This crack propagates into the dam along the dam–foundation boundary. The nucleation of this crack is induced by the stress concentration in this area due to the infinitely rigid foundation. At this time, some partial tensile damage is also observed on several elements along the upstream face.During the next large excursion in the upstream direction, at time, a localized band of damaged elements forms near the downstream change of slope. As this downstream crack propagates toward the upstream direction, it curves down due to the rocking motion of the top block of the dam. The crack at the base of the dam is closed at time by the compressive stresses in this region. This is easily verified by looking at the contour plot of SDEG at time, which clearly shows that the stiffness is recovered on this region, indicating that the crack is closed.When the load is reversed, corresponding to the next excursion in the downstream direction at time, the downstream crack closes and the stiffness is recovered on that region. At this time tensile damage localizes on several elements along the upstream face, leading to the formation of a horizontal crack that propagates toward the downstream crack.As the upper block of the dam oscillates back and forth during the remainder of the earthquake, the upstream and downstream cracks close and open in an alternate fashion. The dam retains its overall structural stability since both cracks are never under tensile stress during the earthquake. The distribution of tensile damage at the end of the earthquake is shown in Figure 2.1.15–8, at time. The contour plot of the stiffness degradation variable indicates that, except at the vicinity of the crack tips, all cracks are closed under compressive stresses and most of the stiffness is recovered. No compressive failure is observed during the simulation. The damage patterns predicted by Abaqus are consistent with those reported by other investigators.Abaqus/Explicit resultsFigure 2.1.15–9shows the distribution of tensile damage at the end of the Abaqus/Explicit simulation. Two major cracks develop during the earthquake, one at the base of the dam and the other at the downstream change of slope. If we compare these results with those from the analysis in Abaqus/Standard (see Figure 2.1.15–8at time), we find that Abaqus/Standard predicted additional damage localization zones on the upstream face of the dam. The differences between the results are due to the effect of the dam–reservoir hydrodynamic interactions, which are included in the Abaqus/Standard simulation via an added-mass user element and are ignored in Abaqus/Explicit. This is easily verified by running an Abaqus/Standard analysis without the added-mass user element. The results from this analysis, shown in Figure 2.1.15–10, are consistent with the Abaqus/Explicit results in Figure 2.1.15–9and confirm that additional damage to the upstream wall occurs when the hydrodynamic interactions are taken into account.Input filesAbaqus/Standard input files1、Frequency analysis of the Koyna dam.*HEADINGKOYNA DAM: NA TURAL FREQUENCY EXTRACTION Units - n, m, sec*PREPRINT, MODEL=YES*NODE1 , 0.00, 0.0021, 70.00, 0.002401, 0.00, 66.502421, 19.25, 66.503601, 0.00, 91.753621, 16.17, 91.753801, 0.00, 103.003821, 14.80, 103.00*NGEN, NSET=NBASE1, 21*NGEN, NSET=NMID2401, 2421*NGEN, NSET=NWL3601, 3621*NGEN, NSET=NTOP3801, 3821*NFILL, BIAS=1.05NBASE, NMID, 24, 100*NFILL, BIAS=0.92NMID, NWL, 12, 100*NFILLNWL, NTOP, 2, 100*ELEMENT, TYPE=CPS4R1, 1, 2, 102, 101*ELGEN, ELSET=DAM1, 20, 1, 1, 38, 100, 100*ELSET, ELSET=WDAM, GENERATE1, 3501, 100*SOLID SECTION, ELSET=DAM, MA TERIAL=CONCRETE 1.0,*MATERIAL, NAME=CONCRETE*ELASTIC3.1027E+10, 0.2*DENSITY2643.0*CONCRETE DAMAGED PLASTICITY36.31*CONCRETE COMPRESSION HARDENING13.0E+6, 0.024.1E+6, 0.001*CONCRETE TENSION STIFFENING, TYPE=DISPLACEMENT2.9E+6 ,01.94393E+6 ,0.0000661851.30305E+6 ,0.000122860.873463E+6 ,0.0001734270.5855E+6 ,0.000220190.392472E+6 ,0.0002647180.263082E+6 ,0.0003080880.176349E+6 ,0.000351050.11821E+6 ,0.0003941380.0792388E+6 ,0.0004377440.0531154E+6 ,0.000482165*CONCRETE TENSION DAMAGE, TYPE=DISPLACEMENT0 ,00.381217 ,0.0000661850.617107 ,0.000122860.763072 ,0.0001734270.853393 ,0.000220190.909282 ,0.0002647180.943865 ,0.0003080880.965265 ,0.000351050.978506 ,0.0003941380.9867 ,0.0004377440.99177 ,0.000482165*BOUNDARYNBASE, 1,2*STEP, NLGEOMSTEP 1 - GRA VITY LOAD*STATIC1.0E-10, 1.0E-10*DLOADDAM, GRA V, 9.81, 0, -1*END STEP*STEPSTEP 2 - FREQUENCY EXTRACTION*FREQUENCY4,*END STEP2、Seismic analysis of the Koyna dam, including hydrodynamic interactions. *HEADINGKOYNA DAM: SEISMIC ANAL YSISUnits - n, m, sec********************************************* ** Step 1: Gravity load** Step 2: Hydrostatic pressure load** Step 3: Earthquake including hydrodynamic**interactions**** Requires FORTRAN subroutine addedmass_uel,** and amplitude curves koyna_haccel.inp and** koyna_vaccel.inp**** Execution command:** abaqus job=koyna_std user=addedmass_uel********************************************* *PREPRINT, MODEL=YES*NODE1 , 0.00, 0.0021, 70.00, 0.002401, 0.00, 66.502421, 19.25, 66.503601, 0.00, 91.753621, 16.17, 91.753801, 0.00, 103.003821, 14.80, 103.00*NGEN, NSET=NBASE1, 21*NGEN, NSET=NMID2401, 2421*NGEN, NSET=NWL3601, 3621*NGEN, NSET=NTOP3801, 3821*NFILL, BIAS=1.05NBASE, NMID, 24, 100*NFILL, BIAS=0.92NMID, NWL, 12, 100*NFILLNWL, NTOP, 2, 100*NSET, NSET=NOUT1, 3801*ELSET, ELSET=EOUT2320, 2420*ELEMENT, TYPE=CPS4R1, 1, 2, 102, 101*ELGEN, ELSET=DAM1, 20, 1, 1, 38, 100, 100*ELSET, ELSET=WDAM, GENERATE1, 3501, 100*USER ELEMENT, NODES=2, TYPE=U1, PROP=3, COORD=2 1, 2*ELEMENT, TYPE=U110001, 1, 101*ELGEN, ELSET=ADDED_MASS10001, 36, 100, 1*UEL PROPERTY, ELSET=ADDED_MASS91.75, 91.75, 1000.0*SOLID SECTION, ELSET=DAM, MA TERIAL=CONCRETE 1.0,*MATERIAL, NAME=CONCRETE*ELASTIC3.1027E+10, 0.2*DENSITY2643.0*DAMPING, BETA=0.00323*CONCRETE DAMAGED PLASTICITY36.31*CONCRETE COMPRESSION HARDENING13.0E+6, 0.024.1E+6, 0.001*CONCRETE TENSION STIFFENING, TYPE=DISPLACEMENT 2.9E+6 ,01.94393E+6 ,0.0000661851.30305E+6 ,0.000122860.873463E+6 ,0.0001734270.5855E+6 ,0.000220190.392472E+6 ,0.0002647180.263082E+6 ,0.0003080880.176349E+6 ,0.000351050.11821E+6 ,0.0003941380.0792388E+6 ,0.0004377440.0531154E+6 ,0.000482165*CONCRETE TENSION DAMAGE, TYPE=DISPLACEMENT0 ,00.381217 ,0.0000661850.617107 ,0.000122860.763072 ,0.0001734270.853393 ,0.000220190.909282 ,0.0002647180.943865 ,0.0003080880.965265 ,0.000351050.978506 ,0.0003941380.9867 ,0.0004377440.99177 ,0.000482165*BOUNDARYNBASE, 1,2*AMPLITUDE, NAME=HAMP, INPUT=koyna_haccel.inp*AMPLITUDE, NAME=V AMP, INPUT=koyna_vaccel.inp***STEP, NLGEOM, UNSYMM=YESSTEP 1 - GRA VITY LOAD*STATIC1.0E-10, 1.0E-10*DLOADDAM, GRA V, 9.81, 0, -1*OUTPUT, FIELD, V ARIABLE=PRESELECT, FREQ=10*ELEMENT OUTPUTS,PE,LE,PEEQ,PEEQT,DAMAGEC,DAMAGET,SDEG*OUTPUT, HISTORY, V ARIABLE=PRESELECT, FREQ=1*ELEMENT OUTPUT, ELSET=EOUTSP1*NODE OUTPUT, NSET=NOUTU*END STEP***STEP, NLGEOM, UNSYMM=YESSTEP 2 - HYDROSTA TIC LOAD*STATIC1.0E-10, 1.0E-10*DLOADWDAM, HP4, 900067.6, 91.75, 0*END STEP***STEP, INC=2000, NLGEOM, UNSYMM=YESSTEP 3 - EARTHQUAKE*DYNAMIC, HAFTOL=1.0E70.02, 10.0, 1E-15,0.02*CONTROLS,PARAMETERS=FIELD1.E-5*BOUNDARY,TYPE=ACCELERATION,AMPLITUDE=HAMP NBASE,1,1,9.81*BOUNDARY,TYPE=ACCELERATION,AMPLITUDE=V AMPNBASE,2,2,9.81***CONTROLS, ANAL YSIS=DISCONTINUOUS*END STEP3、Seismic analysis of the Koyna dam, not including hydrodynamic interactions. *HEADINGKOYNA DAM: SEISMIC ANAL YSIS(without hydrodynamic interactions)Units - n, m, sec*************************************************** Step 1: Gravity load** Step 2: Hydrostatic pressure load** Step 3: Earthquake** (without hydrodynamic interactions)**** Requires amplitude curves koyna_haccel.inp** and koyna_vaccel.inp**************************************************PREPRINT, MODEL=YES*NODE1 , 0.00, 0.0021, 70.00, 0.002401, 0.00, 66.502421, 19.25, 66.503601, 0.00, 91.753621, 16.17, 91.753801, 0.00, 103.003821, 14.80, 103.00*NGEN, NSET=NBASE1, 21*NGEN, NSET=NMID2401, 2421*NGEN, NSET=NWL3601, 3621*NGEN, NSET=NTOP3801, 3821*NFILL, BIAS=1.05NBASE, NMID, 24, 100*NFILL, BIAS=0.92NMID, NWL, 12, 100*NFILLNWL, NTOP, 2, 100*NSET, NSET=NOUT1, 3801*ELSET, ELSET=EOUT2320, 2420*ELEMENT, TYPE=CPS4R1, 1, 2, 102, 101*ELGEN, ELSET=DAM1, 20, 1, 1, 38, 100, 100*ELSET, ELSET=WDAM, GENERATE1, 3501, 100*SOLID SECTION, ELSET=DAM, MA TERIAL=CONCRETE 1.0,*MATERIAL, NAME=CONCRETE*ELASTIC3.1027E+10, 0.2*DENSITY2643.0*DAMPING, BETA=0.00323*CONCRETE DAMAGED PLASTICITY36.31*CONCRETE COMPRESSION HARDENING13.0E+6, 0.024.1E+6, 0.001*CONCRETE TENSION STIFFENING, TYPE=DISPLACEMENT 2.9E+6 ,01.94393E+6 ,0.0000661851.30305E+6 ,0.000122860.873463E+6 ,0.0001734270.5855E+6 ,0.000220190.392472E+6 ,0.0002647180.263082E+6 ,0.0003080880.176349E+6 ,0.000351050.11821E+6 ,0.0003941380.0792388E+6 ,0.0004377440.0531154E+6 ,0.000482165*CONCRETE TENSION DAMAGE, TYPE=DISPLACEMENT0 ,00.381217 ,0.0000661850.617107 ,0.000122860.763072 ,0.0001734270.853393 ,0.000220190.909282 ,0.0002647180.943865 ,0.0003080880.965265 ,0.000351050.978506 ,0.0003941380.9867 ,0.0004377440.99177 ,0.000482165*BOUNDARYNBASE, 1,2*AMPLITUDE, NAME=HAMP, INPUT=koyna_haccel.inp*AMPLITUDE, NAME=V AMP, INPUT=koyna_vaccel.inp***STEP, NLGEOM, UNSYMM=YESSTEP 1 - GRA VITY LOAD*STATIC1.0E-10, 1.0E-10*DLOADDAM, GRA V, 9.81, 0, -1*OUTPUT, FIELD, V ARIABLE=PRESELECT, FREQ=10*ELEMENT OUTPUTS,PE,LE,PEEQ,PEEQT,DAMAGEC,DAMAGET,SDEG*OUTPUT, HISTORY, V ARIABLE=PRESELECT, FREQ=1*ELEMENT OUTPUT, ELSET=EOUTSP1*NODE OUTPUT, NSET=NOUTU*END STEP***STEP, NLGEOM, UNSYMM=YESSTEP 2 - HYDROSTA TIC LOAD*STATIC1.0E-10, 1.0E-10*DLOADWDAM, HP4, 900067.6, 91.75, 0*END STEP***STEP, INC=2000, NLGEOM, UNSYMM=YESSTEP 3 - EARTHQUAKE*DYNAMIC, HAFTOL=1.0E70.02, 10.0, 1E-15,0.02*CONTROLS,PARAMETERS=FIELD1.E-5*BOUNDARY,TYPE=ACCELERATION,AMPLITUDE=HAMP NBASE,1,1,9.81*BOUNDARY,TYPE=ACCELERATION,AMPLITUDE=V AMP NBASE,2,2,9.81***CONTROLS, ANAL YSIS=DISCONTINUOUS*END STEP4、Transverse ground acceleration record.** Horizontal accelerogram of Koyna earthquake0.00000E+00, 0.00000E+00, 0.10000E-01, 0.12650E-01, 0.20000E-01, 0.11990E-01, 0.30000E-01, 0.11330E-01, 0.40000E-01, 0.10670E-01, 0.50000E-01, 0.98100E-02, 0.60000E-01, 0.23900E-02, 0.70000E-01,-0.50200E-02, 0.80000E-01,-0.12430E-01, 0.90000E-01,-0.18890E-01, 0.10000E+00,-0.10440E-01, 0.11000E+00,-0.19800E-02, 0.12000E+00, 0.64700E-02, 0.13000E+00, 0.88000E-03, 0.14000E+00,-0.14880E-01, 0.15000E+00,-0.28860E-01, 0.16000E+00,-0.24910E-01, 0.17000E+00,-0.20970E-01, 0.18000E+00,-0.17020E-01, 0.19000E+00,-0.13070E-01, 0.20000E+00,-0.91200E-02, 0.21000E+00,-0.51700E-02, 0.22000E+00,-0.12200E-02, 0.23000E+00,-0.17500E-02, 0.24000E+00,-0.47800E-02, 0.25000E+00,-0.78200E-02, 0.26000E+00,-0.10860E-01, 0.27000E+00,-0.13900E-01, 0.28000E+00,-0.16940E-01, 0.29000E+00,-0.17770E-01, 0.30000E+00,-0.85700E-02, 0.31000E+00, 0.64000E-03, 0.32000E+00, 0.98500E-02, 0.33000E+00, 0.19060E-01, 0.34000E+00, 0.24360E-01, 0.35000E+00, 0.14960E-01, 0.36000E+00, 0.55600E-02, 0.37000E+00,-0.38300E-02, 0.38000E+00,-0.12000E-02, 0.39000E+00, 0.58800E-02, 0.40000E+00, 0.12970E-01, 0.41000E+00, 0.55300E-02, 0.42000E+00,-0.16410E-01, 0.43000E+00,-0.38360E-01, 0.44000E+00,-0.37050E-01, 0.45000E+00,-0.14260E-01, 0.46000E+00, 0.85300E-02, 0.47000E+00, 0.31320E-01, 0.48000E+00, 0.31950E-01, 0.49000E+00, 0.26700E-01, 0.50000E+00, 0.25790E-01, 0.51000E+00, 0.35010E-01, 0.52000E+00, 0.44240E-01, 0.53000E+00, 0.34400E-01, 0.54000E+00, 0.20370E-01, 0.55000E+00, 0.63400E-02, 0.56000E+00,-0.60100E-02, 0.57000E+00,-0.13100E-02, 0.58000E+00, 0.33800E-02, 0.59000E+00, 0.80800E-02, 0.60000E+00, 0.64700E-02, 0.61000E+00, 0.82000E-03, 0.62000E+00, 0.11550E-01, 0.63000E+00, 0.24730E-01, 0.64000E+00, 0.37900E-01, 0.65000E+00, 0.51080E-01, 0.66000E+00, 0.50240E-01, 0.67000E+00, 0.47850E-01, 0.68000E+00, 0.39170E-01, 0.69000E+00,-0.25300E-02, 0.70000E+00,-0.44230E-01, 0.71000E+00,-0.75110E-01, 0.72000E+00,-0.56710E-01, 0.73000E+00,-0.38300E-01, 0.74000E+00,-0.19900E-01, 0.75000E+00,-0.10370E-01, 0.76000E+00,-0.11690E-01, 0.77000E+00,-0.13010E-01, 0.78000E+00, 0.94780E-01, 0.79000E+00, 0.71310E-01, 0.80000E+00, 0.43670E-01, 0.81000E+00, 0.16030E-01, 0.82000E+00,-0.11600E-01, 0.83000E+00,-0.39240E-01, 0.84000E+00,-0.18150E-01, 0.85000E+00, 0.29400E-02, 0.86000E+00, 0.24030E-01, 0.87000E+00, 0.31490E-01, 0.88000E+00, 0.39100E-02, 0.89000E+00,-0.23670E-01, 0.90000E+00,-0.20300E-02, 0.91000E+00, 0.32700E-01, 0.92000E+00, 0.63640E-01, 0.93000E+00, 0.35200E-01, 0.94000E+00, 0.67600E-02, 0.95000E+00,-0.19350E-01, 0.96000E+00,-0.14620E-01, 0.97000E+00,-0.98900E-02, 0.98000E+00,-0.51500E-02, 0.99000E+00,-0.42000E-03, 0.10000E+01, 0.43100E-02, 0.10100E+01, 0.90400E-02, 0.10200E+01, 0.34000E-02, 0.10300E+01,-0.39400E-02, 0.10400E+01, 0.10820E-01, 0.10500E+01, 0.38540E-01, 0.10600E+01, 0.43160E-01, 0.10700E+01, 0.11640E-01, 0.10800E+01,-0.19880E-01, 0.10900E+01,-0.40000E-03, 0.11000E+01, 0.24740E-01, 0.11100E+01, 0.42490E-01, 0.11200E+01, 0.24100E-01, 0.11300E+01, 0.57100E-02, 0.11400E+01, 0.16100E-02, 0.11500E+01, 0.42400E-02, 0.11600E+01, 0.37100E-02, 0.11700E+01,-0.94600E-02, 0.11800E+01,-0.22640E-01, 0.11900E+01,-0.26450E-01, 0.12000E+01, 0.29000E-02, 0.12100E+01, 0.32250E-01, 0.12200E+01, 0.61600E-01, 0.12300E+01, 0.48040E-01, 0.12400E+01, 0.34050E-01, 0.12500E+01, 0.20060E-01, 0.12600E+01, 0.60700E-02, 0.12700E+01,-0.79200E-02, 0.12800E+01,-0.21910E-01, 0.12900E+01,-0.16220E-01, 0.13000E+01,-0.46500E-02, 0.13100E+01, 0.69300E-02, 0.13200E+01, 0.18500E-01, 0.13300E+01, 0.30070E-01, 0.13400E+01, 0.30620E-01, 0.13500E+01, 0.29910E-01, 0.13600E+01, 0.32540E-01, 0.13700E+01, 0.35170E-01, 0.13800E+01, 0.20130E-01, 0.13900E+01, 0.14700E-02, 0.14000E+01,-0.17190E-01, 0.14100E+01,-0.68100E-02, 0.14200E+01, 0.86900E-02, 0.14300E+01, 0.21460E-01, 0.14400E+01, 0.12060E-01, 0.14500E+01, 0.10380E-01, 0.14600E+01, 0.21880E-01, 0.14700E+01, 0.27750E-01, 0.14800E+01, 0.24450E-01, 0.14900E+01, 0.21150E-01, 0.15000E+01, 0.26130E-01, 0.15100E+01, 0.43530E-01, 0.15200E+01, 0.60940E-01, 0.15300E+01, 0.72470E-01, 0.15400E+01, 0.55300E-01, 0.15500E+01, 0.38140E-01, 0.15600E+01, 0.20980E-01, 0.15700E+01, 0.38200E-02, 0.15800E+01,-0.13340E-01, 0.15900E+01,-0.30510E-01, 0.16000E+01,-0.30440E-01, 0.16100E+01,-0.10160E-01, 0.16200E+01, 0.10130E-01, 0.16300E+01, 0.30420E-01, 0.16400E+01, 0.28080E-01, 0.16500E+01, 0.25280E-01, 0.16600E+01, 0.22480E-01, 0.16700E+01, 0.19680E-01, 0.16800E+01, 0.81130E-01, 0.16900E+01, 0.16287E+00, 0.17000E+01, 0.15773E+00, 0.17100E+01, 0.72390E-01, 0.17200E+01,-0.12940E-01, 0.17300E+01,-0.98280E-01, 0.17400E+01,-0.18361E+00, 0.17500E+01,-0.26895E+00,0.17600E+01,-0.18618E+00, 0.17700E+01,-0.63990E-01, 0.17800E+01, 0.58200E-01, 0.17900E+01, 0.16639E+00, 0.18000E+01, 0.11351E+00, 0.18100E+01, 0.60620E-01, 0.18200E+01, 0.77400E-02, 0.18300E+01,-0.45150E-01, 0.18400E+01,-0.34850E-01, 0.18500E+01,-0.13400E-01, 0.18600E+01, 0.80600E-02, 0.18700E+01,-0.26710E-01, 0.18800E+01,-0.95930E-01, 0.18900E+01,-0.11861E+00, 0.19000E+01,-0.68500E-01, 0.19100E+01,-0.18390E-01, 0.19200E+01,-0.37100E-02, 0.19300E+01,-0.26700E-02, 0.19400E+01, 0.26890E-01, 0.19500E+01, 0.49190E-01, 0.19600E+01, 0.36010E-01, 0.19700E+01, 0.30160E-01, 0.19800E+01, 0.27750E-01, 0.19900E+01, 0.25350E-01, 0.20000E+01,-0.46900E-01, 0.20100E+01,-0.12281E+00, 0.20200E+01,-0.98920E-01, 0.20300E+01,-0.34250E-01, 0.20400E+01, 0.30420E-01, 0.20500E+01, 0.95080E-01, 0.20600E+01, 0.15975E+00, 0.20700E+01, 0.18416E+00, 0.20800E+01, 0.87810E-01, 0.20900E+01,-0.85500E-02, 0.21000E+01,-0.10490E+00, 0.21100E+01,-0.20126E+00, 0.21200E+01,-0.17064E+00, 0.21300E+01,-0.10209E+00, 0.21400E+01,-0.66120E-01, 0.21500E+01,-0.10992E+00, 0.21600E+01,-0.14704E+00, 0.21700E+01,-0.57080E-01, 0.21800E+01,-0.39630E-01, 0.21900E+01,-0.79160E-01, 0.22000E+01,-0.11869E+00, 0.22100E+01,-0.15822E+00, 0.22200E+01,-0.83860E-01, 0.22300E+01, 0.21156E+00, 0.22400E+01, 0.35435E+00, 0.22500E+01, 0.21368E+00, 0.22600E+01, 0.73000E-01, 0.22700E+01,-0.67670E-01, 0.22800E+01,-0.20835E+00, 0.22900E+01,-0.24427E+00, 0.23000E+01,-0.10928E+00, 0.23100E+01, 0.25710E-01, 0.23200E+01, 0.16069E+00, 0.23300E+01, 0.16925E+00, 0.23400E+01, 0.10973E+00, 0.23500E+01, 0.50210E-01, 0.23600E+01,-0.93100E-02, 0.23700E+01,-0.68830E-01, 0.23800E+01,-0.71660E-01, 0.23900E+01, 0.68000E-03, 0.24000E+01, 0.73020E-01, 0.24100E+01, 0.14535E+00, 0.24200E+01, 0.11531E+00, 0.24300E+01, 0.79880E-01, 0.24400E+01, 0.44450E-01, 0.24500E+01, 0.90200E-02, 0.24600E+01, 0.20877E+00, 0.24700E+01, 0.20674E+00, 0.24800E+01, 0.12830E+00, 0.24900E+01, 0.49860E-01, 0.25000E+01,-0.28580E-01, 0.25100E+01,-0.10702E+00, 0.25200E+01,-0.11965E+00, 0.25300E+01,-0.69050E-01, 0.25400E+01,-0.18440E-01, 0.25500E+01, 0.13010E-01, 0.25600E+01,-0.47700E-02, 0.25700E+01,-0.22540E-01, 0.25800E+01,-0.40320E-01, 0.25900E+01,-0.58100E-01, 0.26000E+01,-0.14990E-01, 0.26100E+01, 0.16363E+00, 0.26200E+01, 0.15271E+00, 0.26300E+01, 0.10019E+00, 0.26400E+01, 0.47660E-01, 0.26500E+01,-0.48600E-02, 0.26600E+01,-0.57380E-01, 0.26700E+01,-0.10991E+00, 0.26800E+01,-0.16243E+00, 0.26900E+01,-0.21495E+00, 0.27000E+01,-0.26748E+00, 0.27100E+01,-0.27359E+00, 0.27200E+01,-0.12434E+00, 0.27300E+01, 0.24910E-01, 0.27400E+01, 0.13502E+00, 0.27500E+01, 0.23340E-01, 0.27600E+01,-0.88350E-01, 0.27700E+01,-0.20004E+00, 0.27800E+01,-0.31172E+00, 0.27900E+01,-0.25999E+00, 0.28000E+01,-0.12776E+00, 0.28100E+01, 0.44700E-02, 0.28200E+01, 0.13669E+00, 0.28300E+01, 0.26892E+00, 0.28400E+01, 0.25423E+00, 0.28500E+01, 0.17658E+00, 0.28600E+01, 0.98930E-01, 0.28700E+01, 0.21280E-01, 0.28800E+01,-0.56370E-01, 0.28900E+01,-0.53320E-01, 0.29000E+01,-0.20430E-01, 0.29100E+01, 0.12460E-01, 0.29200E+01, 0.45350E-01, 0.29300E+01, 0.78240E-01, 0.29400E+01, 0.11113E+00, 0.29500E+01, 0.14402E+00, 0.29600E+01, 0.17691E+00, 0.29700E+01, 0.20980E+00, 0.29800E+01, 0.19554E+00, 0.29900E+01, 0.65860E-01, 0.30000E+01,-0.63820E-01, 0.30100E+01,-0.19351E+00, 0.30200E+01,-0.13931E+00, 0.30300E+01,-0.41970E-01, 0.30400E+01, 0.55360E-01, 0.30500E+01, 0.15270E+00, 0.30600E+01, 0.25003E+00, 0.30700E+01, 0.32433E+00, 0.30800E+01, 0.19131E+00, 0.30900E+01, 0.58290E-01, 0.31000E+01,-0.74730E-01, 0.31100E+01,-0.20774E+00, 0.31200E+01,-0.34076E+00, 0.31300E+01,-0.47378E+00, 0.31400E+01,-0.30489E+00, 0.31500E+01,-0.94830E-01, 0.31600E+01, 0.11523E+00, 0.31700E+01, 0.27682E+00, 0.31800E+01, 0.18387E+00, 0.31900E+01, 0.90930E-01, 0.32000E+01,-0.20100E-02, 0.32100E+01,-0.94950E-01, 0.32200E+01,-0.18789E+00, 0.32300E+01,-0.28083E+00, 0.32400E+01,-0.37377E+00, 0.32500E+01,-0.19456E+00, 0.32600E+01, 0.10129E+00, 0.32700E+01, 0.25356E+00, 0.32800E+01,-0.10327E+00, 0.32900E+01,-0.75620E-01, 0.33000E+01,-0.36080E-01, 0.33100E+01,-0.35800E-01, 0.33200E+01,-0.76360E-01, 0.33300E+01,-0.11692E+00, 0.33400E+01,-0.15748E+00, 0.33500E+01,-0.19804E+00, 0.33600E+01,-0.23861E+00, 0.33700E+01,-0.24706E+00, 0.33800E+01,-0.17296E+00, 0.33900E+01,-0.98850E-01, 0.34000E+01,-0.24750E-01, 0.34100E+01, 0.49360E-01, 0.34200E+01, 0.12346E+00, 0.34300E+01, 0.19049E+00, 0.34400E+01, 0.14661E+00, 0.34500E+01, 0.10272E+00, 0.34600E+01, 0.58840E-01, 0.34700E+01, 0.14960E-01, 0.34800E+01,-0.28920E-01, 0.34900E+01,-0.72810E-01, 0.35000E+01,-0.11669E+00, 0.35100E+01,-0.16057E+00,。

基于ABAQUS的水平成层场地地震反应无限元分析

右图曲线坐标的点来给ＡＡＵ动力反应计算提供反应谱曲线，ＢＱＳ计算时间１，０秒在底部基岩处输入地震波进行动力响应计算．
“ ｌ！竺竺
｛ｌ
『ｌｌＩｌＪｆｊ
平面应变单元（Ｐ４，共２９个结点．有限元计算区域两侧设置３ＣＥＲ）一８在４个４结点线性平面应变无限
单元（ＩＰ４与有限单元进行耦合，ＣＮＥ）如图３１ —．地震波选用印度的Ｋｙａｏｎ重力坝地震时记录的水平向加速度时程谱，ＡＡＵ在ＢＱＳ的ｉ文件里用ｎｐ
远处不能相交．
［收稿日］０１１２期２１ — ０－０
［作者简介］琦（９２一，，李１８）男山东泰安人，泰山学院建筑与机械工程系助教
第６期
李
琦：于ＡＡＵ基ＢＱＳ的水平成层场地地震反应无限元分析
７１
已
● ｌ
［中图分类号］Ｔ４７Ｕ５［文献标识码］Ａ［文章编号】１７６２—２９（０１Ｏ０７０５０２１）６— ００— ４
１引言
对于地下结构抗震设计的拟静力计算方法，无论是反应位移法还是反应加速度法，都要先进行一维
土层的地震反应分析．传统的方法都是采用动力有限元法进行分析，它能够出来土体材料的非线性、成
第３３卷第６期
２１年１０１１月
泰山学院学报
ＪＯＵＲＬＯＦＴＩＨＡＵＮＶＥ ⅡＹＮＡＡＳＮＩＲＳ

ABAQUS钢框架结构抗震仿真分析报告

一、引言时程分析法是对结构动力方程直接进行逐步积分求解的一种动力分析方法。

时程分析法将地震波按时段进行数值化后，输入结构体系的振动微分方程，采用直接积分法计算出结构在整个强震时域中的振动状态全过程，给出各个时刻各个杆件的内力和变形。

现已成为多数国家抗震设计规范或规程的分析方法之一。

二、有限元软件ABAQUS简介ABAQUS是美国ABAQUS公司（原名HKS公司．即Hibbitt，Karlsson＆Sorensen,INC．2005年被法国达索公司收购，2007年公司更名为SIMULIA）。

ABAQUS已成为国际上最先进的大型通用有限元力学分析软件之一。

ABAQUS是一套功能强大的进行工程模拟的有限元软件。

其解决问题的范围从相对简单的线性分析到许多复杂的非线性问题。

ABAQUS拥有CAE工业领域最为广泛的材料模型，它可以模拟绝大部分工程材料的线形和非线形行为，可以进行结构的静态和动态分析，如应力、变形、振动、热传导以及对流等。

也可以模拟广泛的材料性能，如金属、橡胶、塑料、弹性泡沫等，而且任何一种材料都可以和任何一种单元或复合材料的层一起用于任何合适的分析类型。

三、模型建立与求解1、PartCreate Part：Name：Ban，3D，Deformable， Shell ，Planar，输入坐标创建一个18X9m的壳部件，作为混凝土楼板部件；Create Part：Name：Zhu，3D，Deformable， Wire ，Planar，输入坐标创建一个长3m线部件，作为柱部件；Create Part：Name：Liang，3D，Deformable， Wire ，Planar，输入坐标创建一个长6X3m，宽4.5X2m的线网部件，作为梁网部件；2、 SectionCreate Material：Name：steel，General，Density 7800；Mechanical，Elasticity，Young’s Modulus 2.1e11，Poisson’ Ratio 0.3；Create Material：Name：concrete，General，Density 2500；Mechanical，Elasticity，Young’s Modulus 3e10，Poisson’ Ratio 0.3。

基于ABAQUS的高混凝土面板堆石坝地震反应三维非线性分析

响应不强烈，但坝顶出现明显的鞭稍效应，需采取相应的抗震工程措施。
关键词： ABAQUS；面板堆石坝；动力响应；非线性有限元；抗震稳定
中国分类号： TV312
文献标识码： A
ABAQUS three-dimensional nonlinear analysis of seismic responses of high CFRD
( ) m Pa
n
（ 1）
其中：
Gmax 为最大剪切模量，K、n
由试验参数确定，σ' m
=
(
σ'1
+
σ'2 3
+ σ'3 ) ，σ'1 、σ'2 、σ'3
为作用于试样的有效主应
力，Pa 为工程大气压。
将
G（ G max
G
为动剪模量）
与动剪应变 γ，及阻尼比 λ 与动剪应变 γ 的动力试验数据进行回归分析，可得到动剪
2 基于 ABAQUS 软件的程序实现及验证
2. 1 程序实现
（ 1）由坝体各单元震前静应力成果，根据式（ 1 ）计算各单元的最大剪切模量 Gmax ，并假定各单元的初始动剪
应变
γ（
γ
= 10
－6）
，从
G G max
～
γ
和
λ
～
γ
曲线插值得到各单元的动剪模量
（ 1. 长江科学院水利部岩土力学与工程重点实验室，武汉 430010； 2. 中国水电顾问集团成都勘测设计研究院，成都 610072； 3. 长江航道规划设计研究院，武汉

Abaqus地震分析的总结

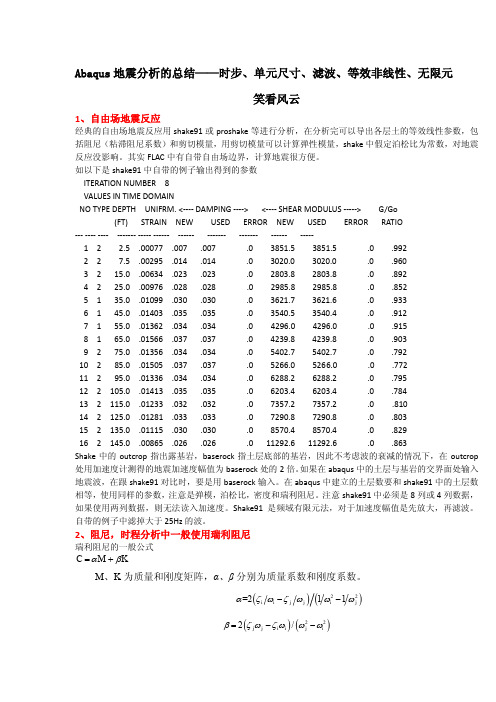
Abaqus 地震分析的总结——时步、单元尺寸、滤波、等效非线性、无限元笑看风云1、自由场地震反应经典的自由场地震反应用shake91或proshake 等进行分析，在分析完可以导出各层土的等效线性参数，包括阻尼（粘滞阻尼系数）和剪切模量，用剪切模量可以计算弹性模量，shake 中假定泊松比为常数，对地震反应没影响。

其实FLAC 中有自带自由场边界，计算地震很方便。

如以下是shake91中自带的例子输出得到的参数ITERATION NUMBER 8VALUES IN TIME DOMAINNO TYPE DEPTH UNIFRM. <---- DAMPING ----> <---- SHEAR MODULUS -----> G/Go(FT) STRAIN NEW USED ERROR NEW USED ERROR RATIO--- ---- ---- ------- ----- ------ ------ ------- ------- ------ -----1 2 2.5 .00077 .007 .007 .0 3851.5 3851.5 .0 .9922 2 7.5 .00295 .014 .014 .0 3020.0 3020.0 .0 .9603 2 15.0 .00634 .023 .023 .0 2803.8 2803.8 .0 .8924 2 25.0 .00976 .028 .028 .0 2985.8 2985.8 .0 .8525 1 35.0 .01099 .030 .030 .0 3621.7 3621.6 .0 .9336 1 45.0 .01403 .035 .035 .0 3540.5 3540.4 .0 .9127 1 55.0 .01362 .034 .034 .0 4296.0 4296.0 .0 .9158 1 65.0 .01566 .037 .037 .0 4239.8 4239.8 .0 .9039 2 75.0 .01356 .034 .034 .0 5402.7 5402.7 .0 .79210 2 85.0 .01505 .037 .037 .0 5266.0 5266.0 .0 .77211 2 95.0 .01336 .034 .034 .0 6288.2 6288.2 .0 .79512 2 105.0 .01413 .035 .035 .0 6203.4 6203.4 .0 .78413 2 115.0 .01233 .032 .032 .0 7357.2 7357.2 .0 .81014 2 125.0 .01281 .033 .033 .0 7290.8 7290.8 .0 .80315 2 135.0 .01115 .030 .030 .0 8570.4 8570.4 .0 .82916 2 145.0 .00865 .026 .026 .0 11292.6 11292.6 .0 .863Shake 中的outcrop 指出露基岩，baserock 指土层底部的基岩，因此不考虑波的衰减的情况下，在outcrop 处用加速度计测得的地震加速度幅值为baserock 处的2倍。

基于ABAQUS的土层地震反应分析

基于ABAQUS的土层地震反应分析
李煜东;李平;孙强强;乔峰;王亮
【期刊名称】《防灾科技学院学报》
【年(卷),期】2016(018)002
【摘要】基于一维波动理论和Spring/Dashpots单元实现了ABAQUS中粘性边界条件的施加,进而对弹性半空间的波源问题进行波动数值模拟,验证了ABAQUS 软件中粘性边界条件的有效性.分别采用ABAQUS软件和RSLEIBM程序计算响嘡台阵3号测井的土层地震反应,并将计算结果与实际记录进行对比分析.结果表明,在地震动不大的情况下,ABAQUS计算结果能够较好地模拟地表地震反应,较RSLEIBM程序计算结果具有更高的准确性.
【总页数】8页(P64-71)
【作者】李煜东;李平;孙强强;乔峰;王亮
【作者单位】防灾科技学院,河北三河065201;防灾科技学院,河北三河065201;防灾科技学院,河北三河065201;中国地震局工程力学研究所,黑龙江哈尔滨150080;中国地震局工程力学研究所,黑龙江哈尔滨150080
【正文语种】中文
【中图分类】P315.9
【相关文献】
1.基于摄动原理的复杂土层地震反应分析的子结构法 [J], 楼梦麟;白建方
2.基于FLAC3D的土层非线性地震反应分析 [J], 苏建锋
3.基于FLAC3D的土层非线性地震反应分析 [J], 苏建锋;
4.基于黏弹性解的土层地震反应分析程序LSSRLI-1和SHAKE2000的对比 [J], 李瑞山;袁晓铭;李程程
5.基于实测记录的土层地震反应分析程序对比研究 [J], 张泽旺;梁发云;梁轩
因版权原因，仅展示原文概要，查看原文内容请购买。

ABAQUS反应谱分析

ABAQUS反应谱分析简介ABAQUS是一种非线性有限元分析软件，广泛应用于工程领域。

反应谱分析是使用ABAQUS进行结构动力学分析的一种方法。

本文将介绍ABAQUS反应谱分析的基本原理，步骤和注意事项。

基本原理反应谱是衡量结构在地震或其他动力荷载作用下的响应的一种方法。

其原理基于结构动力学的理论和地震工程的知识。

反应谱分析的目标是确定结构在不同频率下的最大响应。

ABAQUS使用地震波的加速度数据作为输入，通过有限元分析方法计算结构在不同频率下的响应。

然后通过将结构的加速度响应转换为速度或位移响应，得到最终的结构响应谱。

步骤以下是进行ABAQUS反应谱分析的基本步骤：步骤一：准备模型在进行反应谱分析之前，需要先准备好模型。

这包括定义结构的几何形状、材料特性和边界条件等。

步骤二：定义谱函数在ABAQUS中，可以通过定义谱函数来描述地震波的加速度、速度或位移特性。

谱函数通常是按照指定的地震标准或实测数据来定义的。

步骤三：施加地震荷载在ABAQUS中，可以将定义的谱函数作为地震荷载施加到结构上。

需要指定地震荷载的施加方向和施加位置。

步骤四：设置分析控制参数在进行反应谱分析之前，需要设置一些分析控制参数，如时间步长、模型稳定性控制等。

步骤五：运行分析通过运行ABAQUS的分析命令，开始进行反应谱分析。

ABAQUS将根据定义的谱函数和施加的地震荷载，计算结构在不同频率下的响应，并输出结果。

步骤六：分析结果分析完成后，可以通过查看ABAQUS的分析结果来获取结构在不同频率下的最大响应。

通常会输出加速度、速度和位移等结果。

注意事项在进行ABAQUS反应谱分析时，需要注意以下几点：•模型的准确性：模型的几何形状、材料特性和边界条件等需要准确地定义，以确保分析结果的可靠性。

•谱函数的选择：需要根据具体的地震条件选择合适的谱函数，以保证分析的准确性。

•地震荷载的施加：地震荷载需要按照合适的方向和位置施加到结构上，以确保分析的准确性。

ABAQUS地震反应谱分析

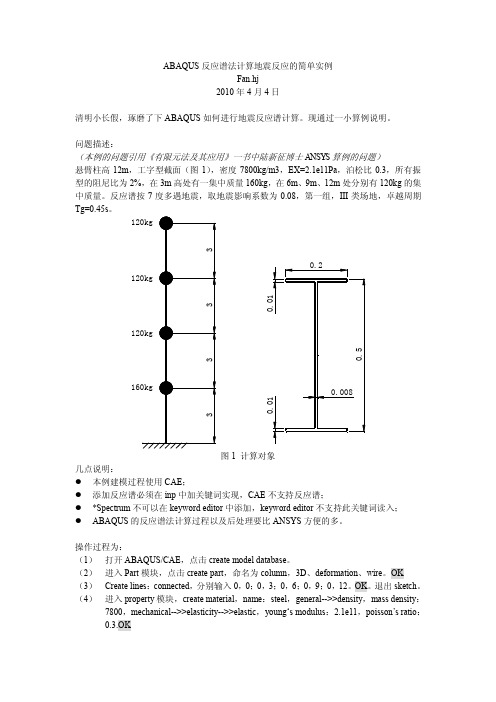
ABAQUS反应谱法计算地震反应的简单实例Fan.hj2010年4月4日清明小长假，琢磨了下ABAQUS如何进行地震反应谱计算。

现通过一小算例说明。

问题描述：（本例的问题引用《有限元法及其应用》一书中陆新征博士ANSYS算例的问题）悬臂柱高12m，工字型截面（图1），密度7800kg/m3，EX=2.1e11Pa，泊松比0.3，所有振型的阻尼比为2%，在3m高处有一集中质量160kg，在6m、9m、12m处分别有120kg的集中质量。

反应谱按7度多遇地震，取地震影响系数为0.08，第一组，III类场地，卓越周期Tg=0.45s。

图1 计算对象几点说明：●本例建模过程使用CAE；●添加反应谱必须在inp中加关键词实现，CAE不支持反应谱；●*Spectrum不可以在keyword editor中添加，keyword editor不支持此关键词读入；●ABAQUS的反应谱法计算过程以及后处理要比ANSYS方便的多。

操作过程为：（1）打开ABAQUS/CAE，点击create model database。

（2）进入Part模块，点击create part，命名为column，3D、deformation、wire。

OK （3）Create lines：connected，分别输入0，0；0，3；0，6；0，9；0，12。

OK。

退出sketch。

（4）进入property模块，create material，name：steel，general-->>density，mass density：7800，mechanical-->>elasticity-->>elastic，young‘s modulus：2.1e11，poisson’s ratio：0.3.OK（5）Create section，name：I，category：beam，type：beam, Continue, create profile, name:I, shape:I, 按图1尺寸输入界面尺寸，ok。

ABAQUS地震波资料

ABAQUS地震波资料提供常⽤的P50%10（50年超越概率10%），⼀般的⼯程设计地震常⽤这个,时间增量0.02秒。

*Amplitude, name=HAMPX0.02, 0.014, 0.04, 0.014, 0.06, 0.014, 0.08, 0.0650.1, 0.014, 0.12, 0.016, 0.14, 0.219, 0.16, 0.130.18, 0.082, 0.2, 0.3, 0.22, 0.583, 0.24, 0.1290.26, -0.263, 0.28, -0.948, 0.3,-0.105, 0.32, -0.5240.34, -0.952, 0.36, 0.088, 0.38, 0.843, 0.4, 1.1520.42, 1.716, 0.44, 2.523, 0.46, 0.07, 0.48, -1.690.5, -0.708, 0.52, -1.42, 0.54,-1.807, 0.56, -1.0910.58, -1.674, 0.6, -2.547, 0.62,-1.639, 0.64, -2.5140.66, -5.463, 0.68, -5.08, 0.7,-5.128, 0.72, -6.9550.74, -7.118, 0.76, -5.805, 0.78,-3.695, 0.8, -1.8710.82, 3.558, 0.84, 6.373, 0.86, 4.406, 0.88, 5.7690.9, 10.47, 0.92, 11.534, 0.94, 10.337, 0.96, 12.440.98, 6.454, 1., 8.596, 1.02, 5.458, 1.04, 6.4031.06, 0.05, 1.08, 1.007, 1.1,-5.859, 1.12, -9.4481.14, -6.851, 1.16, -8.897, 1.18, 12.645, 1.2, 16.451.22, 13.529, 1.24, 12.146, 1.26, 16.093, 1.28, 10.121.3, -9.287, 1.32, 24.022, 1.34, 22.118, 1.36, 21.6571.38, 17.831, 1.4, -1.39, 1.42, 10.005, 1.44, 8.1741.46, 4.502, 1.48, -2.972, 1.5,-7.108, 1.52, -8.635为⽅便⼤家使⽤，已经将其转化为标准的ABAQUS 输⼊格式，数据⽂件是加速度，加速度单位是cm，请在加界中按0.01缩放！在INP中加⼊以下字段：*AMPLITUDE, NAME=HAMPx, INPUT=X.inp*AMPLITUDE, NAME=V AMPy, INPUT=Y.inp*AMPLITUDE, NAME=V AMP, INPUT=Z.inp----------------------------------------------------------------------------------------------*Boundary, op=NEW, amplitude=HAMPx, type=ACCELERA TION“定义的约束集合名”, 1, 1,0.01（红字）场地⼟层反应计算采⽤的输⼊地震波是以地震危险性分析结果得到的基岩加速度峰值和基岩加速度反应谱基岩地震相关反应谱作为⽬标谱，⽤⼈⼯数值模拟⽅法合成得到的，并以此作为场地地震反应计算的输⼊地震波。

ABAQUS地震反应谱分析

ABAQUS钢框架结构抗震仿真分析

地震反应分析

ABAQUS时程分析法计算地震反应的简单实例

abaqus 地下结构抗震 反应位移法

ABAQUS时程分析法计算地震反应的简单实例

ABAQUS钢框架结构抗震仿真分析

ABAQUS软件在基于性能的地震时程分析上的应用

abaqus中地动态分析报告方法

abaqus帮助文档之地震相应计算分析

基于ABAQUS的水平成层场地地震反应无限元分析

ABAQUS钢框架结构抗震仿真分析报告

基于ABAQUS的高混凝土面板堆石坝地震反应三维非线性分析

Abaqus地震分析的总结

基于ABAQUS的土层地震反应分析

ABAQUS反应谱分析

ABAQUS地震反应谱分析

ABAQUS地震波资料

abaqus 地下结构抗震反应位移法