2013年第25届APMO亚太区数学奥林匹克答案详解(英文版)
2013年6年级数学世界奥林匹克竞赛试题与详细答案

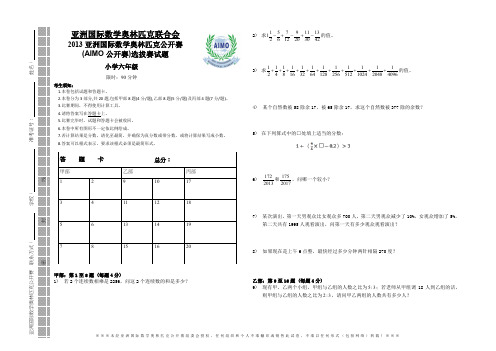
1.设集合M={x∣x2+2x=0,x∈R},N={x∣x2-2x=0,x∈R},则M∪N=A. {0}B. {0,2}C. {-2,0} D {-2,0,2}2.定义域为R的四个函数y=x3,y=2x,y=x2+1,y=2sinx中,奇函数的个数是A. 4B.3C. 2D.13.若复数z满足iz=2+4i,则在复平面内,z对应的点的坐标是A. (2,4)B.(2,-4)C. (4,-2) D(4,2)4.已知离散型随机变量X的分布列为X P1 2 3P则X的数学期望E(X)=A. B. 2 C. D 35.某四棱太的三视图如图1所示,则该四棱台的体积是A.4 B. C.D.66.设m,n是两条不同的直线,α,β是两个不同的平面,下列命题中正确的是A.若α⊥β,m α,n β,则m⊥ n B.若α∥β,m α,n β,则m∥nC.若m⊥ n,m α,n β,则α⊥β D.若m α,m∥n,n∥β,则α⊥β7.已知中心在原点的双曲线C的右焦点为F(3,0),离心率等于,则C的方程是A.= 1 B.= 1 C.= 1 D.= 18.设整数n≥4,集合X={1,2,3……,n}。
令集合S={(x,y,z)|x,y,z∈X,且三条件x<y<z,y<z<x,z<x<y恰有一个成立},若(x,y,z)和(z,w,x)都在s中,则下列选项正确的是A.(y,z,w)∈s,(x,y,w) SB.(y,z,w)∈s,(x,y,w)∈SC. (y,z,w) s,(x,y,w)∈SD. (y,z,w) s,(x,y,w) S二、填空题:本大题共7小题,考生作答6小题,每小题5分,满分30分。
(一)必做题(9~13题)9.不等式x2+x-2<0的解集为。
10.若曲线y=kx+lnx在点(1,k)处的切线平行于x轴,则k= 。
11.执行如图2所示的程序框图,若输入n的值为4,则输出s的值为。
2012年亚太数学奥林匹克初选赛(含答案)

2012年亞太數學奧林匹亞競賽,初選考試試題2012年2月11日說明:將答案標示在答案卡之「解答欄」所標示的列號處。
答錯不倒扣,未完全答對者,不給分。
一、(7分)已知△ABC為直角三角形,其中∠C=90◦,BC=5,另兩邊AB,AC的長度均為正整數。
延長AC到D,使得∠ABD=90◦.則△ABD的外接圓半徑R=1⃝2⃝3⃝/4⃝5⃝(化成最簡分數)。
Ans.169/24.二、(7分)將2n個正整數1,2···,2n任意放置在一個圓周上。
已知,在所有相鄰的三個數中,三個數全為奇數的有a組,三個數恰有兩個為奇數的有b組,三個數中只有一個為奇數的有c組,三個數都是偶數的有d組。
若a=d,則b−c=6⃝7⃝。
Ans.−3三、(7分)已知半徑分別為10與5的兩個圓外切於點P。
試問:點P到這兩個圓的一條外公切線的距離d=8⃝9⃝/10⃝(化成最簡分數)。
Ans.20/3四、對所有兩兩相異的n個正整數a1,a2,···,a n,則在形如t1a1+t2a2+···+t n a n(其中t i為1或−1,i=1,2,···,n)的整數中,必存在S n個不同的整數。
試問(1)(2分)S10=11⃝12⃝Ans.56(2)(5分)S100=13⃝14⃝15⃝16⃝Ans.5051五、已知二元多項式f(x,y),滿足下列條件(i)對任意實數x,f(x,0)=1,(ii)對任意實數x,y,z,f(f(x,y),z)=f(z,xy)+z.試問(1)(2分)f(2012,1)=17⃝18⃝19⃝20⃝Ans.2013(2)(5分)f(2012,2)=21⃝22⃝23⃝24⃝Ans.4025。
2013AIMO亚洲国际数学奥林匹克公开赛选拔赛试题小学六年级
11) 甲、乙、丙三人,一个姓张,一个姓李和一个姓王,他们一个是银行职员,一个是计算机 程序员,一个是秘书。又知甲既不是银行职员也不是秘书;丙不是秘书;张不是银行职员; 姓王的不是乙,也不是丙。问:甲、乙、丙三人分别姓什么?
丙部:第 17 至 20 题 (每题 7 分) 17) 修筑一条高速公路,若甲、乙、丙合作,90 天可完工;若甲、乙、丁合作,120 天可完工; 若丙、丁合作,180 天可完工。若甲、乙合作 72 天后,剩下的工程由甲、乙、丙、丁四人 合作,还需要多少天才可完工?
封
5
6
13
14
19
联系方式:
7 密
8
15
16
20
8)
如果现在是上午 6 点整,最快经过多少分钟两针相隔 270 度?
亚洲国际数学奥林匹克公开赛
甲部:第 1 至 8 题 (每题 4 分) 1) 若 2 个连续数相乘是 2256,问这 2 个连续数的和是多少?
乙部:第 9 至 16 题 (每题 4 分) 9) 现有甲、乙两个小组,甲组与乙组的人数之比为 5 : 3 ;若老师从甲组调 18 人到乙组的话, 则甲组与乙组的人数之比为 2 : 3 ,请问甲乙两组的人数共有多少人?
5) 在下列算式中的□处填上适当的分数:
答
甲部 线 1
题
卡
乙部 2 9
总分:
丙部 10 17 6)
172 175 和 ,问哪一个较小? 2013 2017
学校:
3
4
11
12
18 7) 某次演出,第一天男观众比女观众多 700 人,第二天男观众减少了 10%,女观众增加了 5%, 第二天共有 1995 人观看演出,问第一天有多少观众观看演出?
2013年四年级亚太初赛

29、若 m 个连续自然数的和是 33,则 m 的所有可能取值的和是 。 30、甲、乙两车同时从 A 地出发开往 B 地。出发的时候,甲车的速度比乙车的速度每小时 快 2.5 千米。10 分钟后,甲车减速了;再过 5 分钟后,乙车也减速了,这时乙车比甲车每 小时慢 0.5 千米。又过了 25 分钟后两车同时到达 B 地。那么甲车当时速度每0 在七进制中表示为 (32)7 ,今天是 2012 年 12 月 21 日,请计算
7 10
(2012) (1221) (?)
15、求能被 4 除余 3 的所有的两位数的和。 16、原有重量为 1,2,4,5,8 克的砝码各一个,后来不慎丢失了其中一个砝码,结果天 平无法称出 12 克的重量(砝码须放在天平的一边) ,失去的砝码重 克。 17、正常的钟每走 10 分钟某挂钟走 11 分钟,小林 8:10 把这个挂钟调到正确的时间,那 么,当这个钟显示 11:50 时的正确时刻是几点几分? 18、甲、乙两地相距 600 千米,快车和慢车分别从甲、乙两地子同发相向而行,10 小时相
遇,快车的速度是慢车的两倍。试问:如果慢车比快车早出发 3 小时,当两车相遇时快车 离甲乙两地中点相距多少千米? 19、在等式△×△×△□=△△△中,□和△表示两个不同的非零数字,△□是两位数,△△ △是三位数,则□+△= 。 20、如图,正方形 ABCD 的各个顶点都落在直角三角形 AEF 的各边上。已知正方形 ABCD 的面积是 24,DE 的长是 4。则线段 BF 的长是 。
2013 年第 24 届亚太小学数学奥林匹克邀请赛上海赛区初赛 四年级组-A 卷
90 分钟 (总分:150 分)
1、 计算: (1×2×3×…×8)÷(1+2+3+…+8) 2、 一个数与 17 的和的 17 倍与 17 的差除以 17,所得的商等于 17,则这个数是 。 3、 学校购置 5 张桌子和 8 个凳子共花费 855 元,桌子的单价比凳子的单价贵 15 元,则桌 子的单价是 元。 4、 盒子中有 5 个球,编号分别为 1,2,3,4,5。每次摸出两个球,将其编号相减(大减 小) ,则差是 的可能性最大。 5、 用 48 个黑色或白色的小正形拼成一个大长方形,已知拼成的长方形外圈用的都是黑色 正方形,那么黑色正方形至少有 个。 6、 小胡做一道计算题:某数乘以 4 再减去 8。小胡把题目看成某数乘以 3 再加上 4。没想 到答案碰对了。那么“某数”是 。 7、 如果一个两位数 ab 乘以 7 等于一个三位数 a0b ,则 ab = 。
2013年普通高等学校招生全国统一考试(广东卷)英语及答案详解

2013年普通高等学校招生全国统一考试(广东卷)英语第一节完型填空Number sense is not the ability to count. It is the ability to recognize a 1 in number. Human beings are born with this ability. 2 , Experiments show that many animas are, too. For example, many birds have good number sense. If a nest has four eggs and you remove one, the bird will not 3 . However, if you remove two, the bird 4 leaves. This means that the bird knows the 5 between two and three.Another interesting experiment showed a bird’s 6 number sense. A man was trying to take a photo of a crow(乌鸦) that had a nest in a tower, but the crow always left when she saw him coming. The bird did not 7 until the man left the tower. The man had an 8 . He took another man with him to the tower. One man left and the other stayed, but they did not 9 the bird. The crow stayed away until the second man left, too. The experiment was 10 with three men and then with four men. But the crow did not return to the nest until all the men were 11 . It was not until five men went into the tower and only four left that they were 12 able to fool the crow.How good is a human’s number sense? It’s not very good. For example, babies about fourteen months old almost always notice if something is taken away from a 13 group. But when the number goes beyond three or four, the children are14 fooled.It seems that number sense is something we have in common with many animals in this world, and that our human 15is not much better than a crow’s.1. A. rise B. pattern C. change D. trend2. A. Importantly B. Surprisingly C. Disappointedly D. Fortunately3. A. survive B. care C. hatch D. notice4. A. generally B. sincerely C. casually D. deliberately5. A. distance B. range C. difference D. interval6. A. amazing B. annoying C. satisfying D. disturbing7. A. relax B. recover C. react D. return8. A. appointment B. excuse C. idea D. explanation9. A. fool B. hurt C. catch D. kill10. A. reported B. repeated C. designed D. approved11. A. confused B. gone C. tired D. drunk12. A. gradually B. luckily C. strangely D. finally13. A. single B. small C. local D. new14. A. seldom B. temporarily C. merely D. often15. A. sight B. nature C. ability D. belief第二节语法填空One day, Nick invited his friends to supper. He was cooking some delicious food in the kitchen. Suddenly, he 16 (find) that he had run out of salt. So Nick called to his son, “Go to the village and buy some salt, but pay a fair price for it: neither too much 17 too little.”His son looked surprised. “I can understand why shouldn’t pay too much, Father, but if I can pay less, 18 not save a bit of money?”“That would be a very 19 (reason) thing to do in a big city, but it could destroy a small village like ours,”Nick said.Nick’s guest, 20 had heard their conversation, asked why they should not buy salt more cheaply if they could. Nick replied, “The only reason a man would sell salt 21 a lower price would be because he was desperate for money. And anyone who took advantage of that situation would be showing a lack of respect 22 the sweat and struggle of the man who worked very hard to produce it.”“But such a small thing couldn’t 23 (possible) destroy a village.”“In the beginning, there was only 24 very small amount of unfairness in the world, but everyone added a little, always 25 (think) that it was only small and not very important, and look where we have ended up today.”第三节阅读理解A.Imagination and fantasy can play an important role in achieving the things we fear. Children know this very well. Fred Epstein, in his book I Make It to Five, tells a story he heard from one of his friends about Tom, a four-year-old boy with a cancer in his back bone. He came through several operations and a lot of pain by mastering his imagination.Tom loved to pretend, and he particularly loved to play superheroes. Dr. Epstein explained that it was actually abrilliant way for his young mind to handle the terrifying and painful life he led.The day before his third trip to the operating room, Tom was terribly afraid. “ Maybe I could go as Superman,” he whispered to his mom. Hearing this, the mother hesitated for while. She has avoided buying the expensive costume(戏装), but finally she agreed.The next day Tom appeared as the powerful Superman, showing off through the hospital halls and coolly waving his hand to the people greeting him along the way. And Tom, with the strength of his fantasy, successfully made it through the operation.The power of imagination need not be reserved for children only. We all have the power to use our fantasies to attempt things we never thought possible, to go through those things that seem impossible, and to achieve what we never believed we could. Just as Dr. Epstein puts it, “If you can dream it, you can do it.”It doesn’t mean that y ou should dress as a superhero for your next job interview. But, next time you are tested in a way that seems impossible, imagine what it would take to overcome it. Become the person you need to become to win over your challenge and do it in your mind first. So, let your imagination run wild, and dare to dream.26. What do we know about Tom?A. He was seriously ill.B. He was a dishonest boy.C. He was crazy about magic.D. He was Dr. Epstein’s patient.27. What can be inferred about Tom’s mother?A. She was a rich lady.B. She refused Tom’s request.C. She wanted Tom to be a superhero.D. She wanted to get Tom through the pain.28. When Tom went for the third operation, he_________.A. pretended to be painfulB. acted like a superheroC. appeared in poor spiritsD. argued with his mother29. In the last paragraph, you are advised________.A. to go through some difficult testsB. to wake up from your wild dreamsC. to become a powerful person in your mindD. to wear expensive clothes for job interviews30. What is the purpose of the passage?A. To tell us an interesting story.B. To help us make right decisions.C. To advise us to care about children.D. To encourage us to use our imagination.B.Some people think that success is only for those with talent or those who grow up in the right family, and others believe that success mostly comes down to luck. I’m not going to say luck, talent, and circumstances don’t come into play because they do. Some people are born into the right family while others are born with great intelligence, and that’s just the reality of how life is.However, to succeed in life, one first needs to set a goal and then gradually make it more practical. And, in addition to that, in order to get really good at something, one needs to spend at least 10,000 hours studying and practicing. To become great at certain things, it’ll require even more time, time that most people won’t put in.This is a big reason why many successful people advise you to do something you love. If you don’t enjoy what you do, it is going to feel like unbearable pain and will likely make you quit well before you ever become good at it.When you see people exhibiting some great skills or having achieved great success, you know that they have put in a huge part of their life to get there at a huge cost. It’s sometimes easy to think they got lucky or they were born with some rare talent, but thinking that way does you no good, and there’s a huge chance that you’re wrong anyway.Whatever you do, if you want to become great at it, you need to work day in and day out, almost to the point of addiction, and over a long period of time. If you’re not willing to put in the time and work, don’t expect to receive any rewards. Consistent, hard work won’t guarantee you the level of success you may want, but it will guarantee that you will become rally good at whatever it is you put all that work into.31. Paragraph 1 mainly talks about__________.A. the reasons for successB. the meaning of successC. the standards of successD. the importance of success32. Paragraph 2, the underlined word that refers to__________.A. being good at somethingB. setting a practical goalC. putting in more timeD. succeeding in life33. Successful people suggest doing what one loves because_____________.A. work makes one feel painB. one tends to enjoy his workC. one gives up his work easilyD. it takes a lot of time to succeed34. What can be infer from Paragraph 4?A. Successful people like to show their great skills.B. People sometimes succeed without luck or talent.C. People need to achieve success at the cost of life.D. It helps to think that luck or talent leads to success.35. What is the main theme of the passage?A. Having a goal is vital to success.B. Being good is different from being great.C. One cannot succeed without time and practice.D. Luck, talent and family help to achieve success.C.One day, when I was working as a psychologist in England, an adolescent boy showed up in my office. It was David. He kept waling up and down restlessly, his face pale, and his hands shaking slightly. His head teacher had referred him to me. “This boy has lost his family,” he wrote. “He is understandably very sad and refuses to talk to others, and I’m very worried about him. Can I help?”I looked at David and showed him to a chair. How could I help him? There are problems psychology doesn’t have the answer to, and which no wards can describe. Sometimes the best thing one can do is to listen openly and sympathetically.The first two times we met, David didn’t say a word. He sat there, only looking up to look at the children’s drawings on the wall behind me. I suggested we play a game of chess. He nodded. After that he played chess with me every Wednesday afternoon---in complete silence and without looking at me. It’s not easy to cheat in chess, but I admit I made sure David won once or twice.Usually, he arrived earlier than agreed, took the chess board and pieces from the shelf and began setting them up before I even got a chance to sit down. It seemed as if he enjoyed my company. But why did he never look at me?“Perhaps he simply needs someone to share his pain with,”I thought. “Perhaps he senses that I respect his suffering.” Some months later, when we were playing chess, he looked up at me suddenly.“It’s your turn,” he said.After that day, David started talking. He got friends in school and joined a bicycle club. He wrote to me a few times, about his biking with some friends, and about his plan to get into university. Now he had really started to live his own life.Maybe I gave David something. But I also learned that one---without any words---can reach out to another person. All it takes is a hug, a shoulder to cry on, a friendly touch, and an ear that listens.36. When he first met the author, David_________.A. felt a little excitedB. walked energeticallyC. looked a little nervousD. showed up with his teacher37. As a psychologist, the author __________.A. was ready to listen to DavidB. was skeptical about psychologyC. was able to describe David’s problemD. was sure of handling David’s problem38. David enjoyed being with the author because he____________.A. wanted to ask the author for adviceB. needed to share sorrow with the authorC. liked the children’s drawing in the officeD. beat the author many times in the chess game39. What can be inferred about David?A. He recovered after months of treatment.B. He liked biking before he lost his family.C. He went into university soon after starting to talk.D. He got friends in school before he met the author.40. What made David change?A. His teacher’s help.B. The author’s friendship.C. His exchange of letters with the author.D. The author’s silent communication with him.D.While Jennifer was at home taking an online exam for her business law class, a monitor(监控器) a few hundred miles away was watching her every move.Using a web camera equipped in Jennifer’s Los Angeles apartment, the monitor in Phoenix tracked how frequently her eyes moved from the computer screen and listened for the secret sounds of a possible helper in the room. Her Internet access was locked---remotely---to prevent Internet searches, and her typing style was analyzed to make sure she was who she said she was; Did she enter her student number at the same speed as she had in the past? Or was she slowing down?In the battle against cheating, this is the cutting edge and a key to encourage honestly in the booming field of on line education. The technology gives trust to the entire system, to the institution and to online education in general. Only with solid measures against cheating, experts say, can Internet universities show that their exams and diplomas are valid---that students haven’t searched the Internet to get the right answers.Although online classes have existed for more than a decade, the concern over cheating become sharper in the last year with the growth of “open online courses.” Private colleges, public universities and corporations are jumping into the online education field, spending millions of dollars to attract potential students, while also taking steps to help guarantee honesty at a distance.Aside from the web cameras, a number of other high-tech methods are becoming increasingly popular. Among them are programs that check student’s identities using personas information, such as the telephone numbers they once used.Other programs can produce unique exams by drawing on a large list of questions and can recognize possiblecheaters by analyzing whether difficult test questions are answered at he same speed as easy ones. As in many university classes, term papers are scanned against some large Internet data banks for cheating.41. Why was Jennifer watched in an online exam?A. To correct her typing mistakes.B. To find her secrets in the roomC. To prevent her from slowing down.D. To keep her from dishonest behaviors42. The underlined expression cutting edge in Paragraph 3 is closest in meaning to _______.A. advanced techniqueB. sharpening toolC. effective ruleD. dividing line43. For Internet universities, exams and diplomas will be valid if__________.A. they can attract potential studentsB. they can defeat academic cheatingC. they offer students online helpD. they offer many online courses44. Some programs can find out possible cheaters by_________.A. checking the question answering speedB. producing a large number of questionsC. scanning the Internet test questionsD. giving difficult test questions45. Which of the following is the best title of this passage?A. The Advantages of Online ExamsB. The High-tech Methods in Online CoursesC. The Fight against Cheating in Online EducationD. The War against the Booming of Online Education第四节信息匹配A. FREE HEALTH BOOKSVisit www. Health Book now for instant access to easy-to-read summaries of the top books on health, food and fitness emailed to you automatically, for FREE. Call Now 1-866-720-3880B. Ready to use straight from the pack; Low self discharge for long storage period.Super Durable Battery; Wide application range From digital cameras to clocks.C. Save GEICO Big savings look good on you. Find our how much you could be saving on an insurance visit , call 1-800-947-AUTO[2886], or contact your local GEICO office today. See for more details.D. FREE Home Security System! $850 value! Free Wireless Remote Control. Protect your home 1-866-711-3832.E. THINGS ARE LOOKING UP VWCARS Take a little off the top in the all-new V okswagon Beetle Convertible 300-hp turbocharged engine offers plenty of substance behind the style traceable top. /beetleconvertible.F. Global Executive MBA Georgetown Unlverslty & ESADE It’s the global MBA. Graduates receive MBA diplomas from both Georgetown University and ESADE Business School. Discover More Visit: globalexecmba. Com46. I am a journalist working for a local news agency. Since I need to travel a lot, I do care about durability choosing power supply for my products to me? Your timely reply will be highly appreciated.47. My family is new in the town. After settling down, we started looking for a family car. Your new model Beetle Convertible looks so cool. I think your detailed information booklet us know more about this model. Please kindly send it to my email address: jacks@. Many thanks for your help.48. My Beetle Convertible is insured with INC insurance company. The insurance will expire next month. The offer introduced in your advertisement attractive to me. I want to know more about your service. Please kindly email me a relevant information booklet. Your timely reply will be highly appreciated.49. I don’t enjoy my new job as a department manager since I find hard to communicate with and motivate my team members. Hopefully, your diploma course can provide me with professional training on administrative and communicative skills. I look forward to receiving your information booklet and application forms as soon as possible. Please send them to me at jimmys@.50. My family is new in the town. Worrying about community safety, I prefer to secure my house with new technology. Your product sounds like the right choice for me. Could you kindly send your information booklet to my email address: peterw@? Your timely reply will be highly appreciated.第五节基础写作请根据以下信息,写一篇关于移民火星的快讯。
最新亚太数学奥林匹克竞赛试题(2010,2011,2012)

2010年APMO 试题时间:4小时 每题7分1. 已知ABC 中90BAC ∠≠,点O 为ABC 的外心,Γ为BOC 的外接圆.设Γ与线段AB 交于点P (异于B ),与线段AC 交于点Q (异于C ).设ON 为圆Γ的直径,证明四边形APNQ 是平行四边形.2. 若一个正整数能够表示成为km 的形式(其中,m k 均为正整数),则称该数为纯k 次方数.证明对于任意正整数n ,都存在n 个不同的正整数,它们之和是一个纯2009次方数,它们之积是一个纯2010次方数.3. 设n 是一个正整数.n 个人参加某一晚会.晚会中的任意两人要么相互认识,要么相互不认识.若两个互不相识的人,A B ,存在一个人C 与,A B 均相识,则称,A B 为一组有缘组,求这样的有缘组数最大可能值.4. 已知ABC 中,AB BC >,AC BC >,,O H 分别表示ABC 的外心与垂心.设AHC 的外接圆与AB 相交于M (异于A ),AHB 的外接圆与AC 相交于N (异于A ).证明MNH 的外接圆心在OH 上.5. 求所有满足以下条件的函数:f R R →,对于,,x y z R ∀∈,均有 (()()())(()())(2())2()f f x f y f z f f x f y f xy f z f xz yz ++=-+++-.2011年APMO 试题时间:4小时 每题7分1. 证明:存在正整数,,a b c 使得222,,a b c b c a c a b ++++++均为完全平方数.2. 已知五个点12345,,,,A A A A A 在同一平面上且任意三点不共线,求所有角(15)i j k A A A i j k ∠≤<<≤中最小角的最大可能值. 3. 已知ABC 中30BAC ∠= ,ABC ∠的内角平分线、外角平分线与AC 分别交于点1B 、2B ,ACB ∠的内角平分线、外角平分线与AB 分别交于点1C 、2C ,设分别与12B B 、12C C 为直径的圆在ABC 内的交点为P .求证:90BPC ∠= .4. 设n 是一个固定的正奇数.坐标平面上相异的2m +个点011,,,(0,)m P P P m m Z +≥∈满足以下三个条件:(1)01(0,1),(1,)m P P n n ++,(1)i P i m ≤≤的横坐标和纵坐标均是不小于1且不大于n 的整数;(2)对于,0i Z i m ∈≤≤,当i 为偶数时,1i i PP +平行于x 轴;当i 为奇数时,1i i PP +平行于y 轴;(3)对于任意,,0i j Z i j m ∈≤<≤,线段1i i PP +和线段1j j P P +最多相交于1点;求m 的最大可能值.5. 求满足以下两个条件的所有函数:f R R →:(1)M R ∃∈,使得x R ∀∈,都有()f x M <成立;(2)对于任意实数对(,)x y ,都有(())()()()f xf y yf x xf y f xy +=+恒成立.2012年APMO 试题时间:4小时 每题7分1. 设P 为ABC 内的一点,AP 、BP 、CP 的延长线分别与BC 、CA 、AB 交于点D 、E 、F ,若PFA 、PDB 、PEC 的面积均为1,求证:ABC 的面积为6.2. 在一张20122012⨯方格表中的每一格都填上一个不小于0且不大于1的实数,用一条平行于方格表边界的线把方格表分割成两个长方形.假设对于所有的分割方法都至少有一个长方形格子里的数之和不大于1,求这个方格表所有格子里的数之和的最大值。
李耀文——2020EMLO第4题解答

李耀文——2020EMLO第4题解答李耀文老师近期文章2020-09-07 2020爱尔兰数学奥林匹克第3题解2020-09-05 2020法语国家数学奥林匹克平几题解答2020-09-04 2020新西兰数学奥林匹克(第一轮)平几题解答2020-09-03 2020环球城市春季赛A级别初级组第5题解答2020-09-02 2020环球城市春季赛O级别初级组第3题解答2020-07-02 2020年比荷卢数学竞赛第3题解答2020-07-01 2019墨西哥数学奥林匹克模拟第1题解2020-06-30 2020年福建省高中数学竞赛(预赛)第13题另解2020-06-28 2020美国初中在线数学奥林匹克第4题解答2020-06-27 万喜人高联班几何测试(32-1)解答2020-06-24 2019澳大利亚数学奥林匹克平几题解答2020-06-23 2020澳大利亚数学奥林匹克(第一天)平几题解答2020-06-21 2020年亚太地区数学奥林匹第1题解2020-06-18 万喜人高联班几何测试(31-1)解答2020-06-08 万喜人高联班几何测试(30-1)解答2020-06-08 2020环球城市春季赛O级别第2题解答2020-06-07 2020FARMLA团体赛第5题解答2020-06-06 再解2020女子数学锦标赛(巴西)第2、5题2020-06-03 第五届爱尖子数学能力测评试题(加试部分)第1题解答2020-05-31 2010年JBMO预选题(几何部分)解答2020-05-30 三角形等角线的一个新性质2020-05-29 2020年圣彼得堡数学竞赛九年级组城市赛第5题解答2020-05-26 万喜人高联班几何测试(27-1、2)解答2020-05-25 2017年希腊JBMO代表队选拔考试第2题解答2020-05-22 2009年JBMO预选题(几何部分)第5题解答2020-05-18 2020USMCA(超级组)第2题解答2020-05-17 2011年JBMO预选题(几何部分)第1、2题解答2020-05-16 2017年奥地利数学奥林匹克(国家级决赛(第2部分第2天)第5题解答2020-05-14 2017年台湾代表队选拔考试(第二轮)测试3第2题解答2020-05-13 2019几何奥林匹克模拟赛第4题解答2020-05-12 一道根源杯几何试题的另解2020-05-11 2020FAKE USAJM第1题解答2020-05-10 2020年比荷卢数学竞赛第3题解答2020-05-09 第83届莫斯科数学奥林匹克(2020)十年级第4题解答2020-05-04 2017年荷兰IMO代表队选拔考试(第一题)第4题解答2020-05-01 2020加拿大冬令营模拟奥林匹克第1题解答2020-04-30 万喜人高联班几何测试(14)解答2020-04-28 2020年欧洲女子奥林匹克数学竞赛第5题解答2020-04-27 万喜人高联班几何测试(23-3)解答2020-04-25 万喜人高联班几何测试(23-2)解答2020-04-24 万喜人高联班几何测试(22-1、2)解答2020-04-14 再解竞赛生每日一题2212020-04-13 一道第59届IMO预选几何题解2020-04-12 也解2019-2020 SDPC冬季赛第8题2020-04-11 2020法国JBMO代表队选拔考试(第一轮)平几题解答2020-04-10 2020年1月根源杯MO邀请赛平几试题的纯几何证法2020-04-09 第一届SAFEST奥林匹克几何题解答2020-04-07 2020拉脱维亚IMO代表队选拔考试(第一轮)第5题解答2020-04-06 第30届亚太地区数学奥林匹克平面几何题解2020-04-04 第二届国际大都市竞赛(数学)第1题别解2020-04-03 2020年3月根源杯几何题另解2020-04-02 2020摩尔多瓦IMO代表队选拔考试平面几何题解2020-04-01 2020高加索地区数学奥林匹克几何题解2020-03-21 2020年科哈佛-麻省数学竞赛春季赛(团体赛)第3题解2020-03-29 2020欧洲女子数学奥林匹克土耳其代表队选拔赛(第一天)第1题解答2020-03-26 2019罗马尼亚IMO代表队选拔考试(第五次)几何题解2020-03-25 2018希腊国家队选拔考试第2题解答2020-03-24 加拿大CRUX杂志问题(4401、4415、4440)解答2020-03-23 2018拉普拉塔河学奥林匹克几何题另解2020-03-22 2019墨西哥国家数学奥林匹克第2题解2020-03-20 2019年第21届菲律宾数学奥林匹克第4题解答2020-03-19 再解2016年山大附中高一数学竞赛自测试暨西部选拔赛平几题2020-03-15 2020欧洲女子数学奥林匹克日本代表队选拔考试第3题解答2020-03-14 2020欧洲女子数学奥林匹克美国代表队选拔考试第4题解答2020-03-11 第41届环球城市数学竞赛(2019)秋季赛平几题解2020-03-09 再解第41届环球城市数学竞赛(2019)秋季赛平几题2020-03-08 2019土耳其初中数学奥林匹克第3题解答2020-03-07 2019-2020英国数学奥林匹克(第一轮)第3题另解2020-03-06 一道2012美国国家代表队IMO选拔考试题另解2020-03-01 加拿大杂志CRUX问题4518解答2020-02-29 2020日本数学奥林匹克决赛第2题解答2020-02-28 两道2018年白斯数学奥林匹克试题的解答2020-02-26 加拿大杂志CRUX问题4503解答2020-02-25 2019摩尔多瓦IMO代表队选拔考试第5题的解答2020-02-24 加拿大杂志CRUX问题4505解答2020-02-22 2019伊朗数学奥林匹克第三轮几何部分第2题的另解2020-02-21 2019年波罗的海地区(波兰)数学奥林匹克第14题的三角证法2020-02-20 2018年马来MO提高组B类题目第1题解答2020-02-18 2019南非数学奥林匹克第3题的解答2020-02-17 2019年波罗的海地区(波兰)数学奥林匹克第11题的纯几何解法2020-02-14 一道第21届地中海地区数学奥林匹克(平几)试题的另解2020-02-13 第45届俄罗斯数学奥林匹克决赛(十、十一年级)第4题的另解2020-02-12 第18届中国女子数学奥林匹克第一题的另解。
2013年24届亚太小学数学奥林匹克邀请赛上海赛区五年级组决赛详解

9、小王和小张从 A 学校到 B 学校,小王以每分钟 90 米的速度步行, 10 分钟后小张骑车, 以分钟 240 米的速度从 A 学校到 B 学校,经过()分钟后小张追上了小王。 【分析】 90 10 (240 90) 6 ,故 6 分钟后小张追上小王。
10、甲乙丙分别教语文、数学、英语,现知道:( 1)丙比英语老师大,( 2)甲与数学老 师不同岁;( 3)数学老师比乙年龄小。那么英语老师是()。 【分析】由于甲、乙均与数学老师不同岁,所以数学老师是丙,而丙比英语老师大,又比乙 小,所以乙不是英语老师,是语文老师,英语老师是甲。 11、利用“ +、-、*、/及添加()计算 9、9、15、15,使其结果为 24” ,请写出其表示方 式()。 【分析】 (15 15 9) 9 24 。
2013 年第 24 届亚太小学数学奥林匹克邀请赛 上海赛区决赛(五年级) 1、计算: (98765432.1)2 98765432 98765432.2 =()。
2 【分析】原式 (98765432.1) (98765432.1 0.1) (98765432.1 1) 0.
1 30 6 90 ,得 SDFC 270 90 180 , 2
4 2
2 4
1
4 2 3 3 2 1 2 4 1 3 1 2 3 4 3 1 4 2 1 2
1 ? 2 3
4
2
3
2
【分析】由外面的 4 可得,这列为 1、 2、3、4 ,由外面的 1 可得,该行或列第一个被看到 的是 4,由第一列下方的 3,结合第一列第一个数为 4 ,可知第一列第三个数是 1, 又由第四行右边的 3,结合该行第 2 个数 4 可知,该行的第 3 个数不能是 1 ,于是, 第 4 个数是 1,此时第 2 行第 1 个数可以填了,是 3,于是第 2 行第 3 个数是 1, 于是,第 4 行第 1 个数是 2,第 4 行第 3 个数是 3,此时可知“?”处是 2,具体 填写见下图
25届奥林匹克试题及解答

第25届全国中学生物理竞赛预赛卷一、选择题.本题共6小题.每小题6分.在每小题给出的 4 个选项中,有的小题只有一项是正确的.有的小题有多项是正确的.把正确选项前面的英文字母写在每小题后面的方括号内.全部选对的得6分.选对但不全的得3分.有选错或不答的得O分.1.如图所示,两块固连在一起的物块a和b ,质量分别为m a和m b,放在水平的光滑桌面上.现同时施给它们方向如图所示的推力F a和拉力F b,已知F a>F b,则a对b的作用力A.必为推力B.必为拉力C.可能为推力,也可能为拉力D.可能为零[ ]2.用光照射处在基态的氢原子,有可能使氢原子电离.下列说法中正确的是A.只要光的光强足够大,就一定可以使氢原子电离B.只要光的频率足够高,就一定可以使氢原子电离C.只要光子的能量足够大,就一定可以使氢原子电离D.只要光照的时间足够长,就一定可以使氢原子电离3.如图所示,一U形光滑导轨串有一电阻R,放置在匀强的外磁场中,导轨平面与磁场方向垂直。
一电阻可忽略不计但有一定质量的金属杆ab跨接在导轨上,可沿导轨方向平移。
现从静止开始对ab杆施以向右的恒力F,若忽略杆和U形导轨的自感,则在杆运动过程中,下列哪种说法是正确的?A.外磁场对载流杆ab的作用力对ab杆做功,但外磁场的能量是不变的B.外力F的功总是等于电阻R上消耗的功C.外磁场对载流杆ab作用力的功率与电阻R上消耗的功率两者的大小是相等的D.电阻R 上消耗的功率存在最大值4.如图所示,放置在升降机地板上的盛有水的容器中,插有两根相对容器的位置是固定的玻璃管a和b,管的上端都是封闭的,下端都是开口的.管内被水各封有一定质量的气体.平衡时,a管内的水面比管外低,b管内的水面比管外高.现令升降机从静止开始加速下降,已知在此过程中管内气体仍被封闭在管内,且经历的过程可视为绝热过程,则在此过程中A.a 中气体内能将增加,b中气体内能将减少B.a 中气体内能将减少,b中气体内能将增加C.a 、b中气体内能都将增加D.a 、b中气体内能都将减少5.图示为由粗细均匀的细玻璃管弯曲成的“双U形管”, a 、b 、c 、d为其四段竖直的部分,其中a 、d上端是开口的,处在大气中.管中的水银把一段气体柱密封在b、c 内,达到平衡时,管内水银面的位置如图所示.现缓慢地降低气柱中气体的温度,若c中的水银面上升了一小段高度△h ,则A.b中的水银面也上升△hB.b中的水银面也上升,但上升的高度小于△hC.气柱中气体压强的减少量等于高为△h的水银柱所产生的压强D.气柱中气体压强的减少量等于高为2△h的水银柱所产生的压强6.图中L是绕在铁心上的线圈,它与电阻R、R0 、电键和电池E可构成闭合回路.线圈上的箭头表示线圈中电流的正方向,当电流的流向与箭头所示的方向相同时,该电流为正,否则为负.电键K1和K2都处在断开状态.设在t=0时刻,接通电键K1,经过一段时间,在:t=t l 时刻,再接通电键K2,则能较正确地表示L中的电流I随时间t变化的图线是下面给出的四个图中的哪个图?A.图l B.图2 C.图3 D.图4二、填空题和作圈题.把答案填在题中的横线上或把图画在题指定的地方.只要给出结果.不需写出求得结果的过程.7.(8分)为了估算水库中水的体积,可取一瓶无毒的放射性同位素的水溶液,测得瓶内溶液每分钟衰变6×107次,已知这种同位素的半衰期为2天.现将这瓶溶液倒人水库,8 天后可以认为溶液己均匀分布在水库中,这时取1.0m3水库中的水样,测得水样每分钟衰变20次.由此可知水库中水的体积约为m3。
2012年亚太小学数学奥林匹克第一回合详解

2012年亚太小学奥林匹克第一回合2012年亚太小学奥林匹克第一回合2小时(总分:150分) 2008年4月14日 上午9:0011:00-(注意事项)1 尽量解答所有问题。
2 不准使用数学用表或计算器。
3 答案请另填写在所提供的第一回合的作答卷上。
4 只有正确答案才能得分。
第一题至第十题,每题4分 第十一题至第二十题,每题5分 第二十一题至第三十题,每题6分【第1题】计算:299992999299299++++。
【分析与解】 计算,凑整。
()()()()2999929992992993000013000130013019++++=-+-+-+-+ ()()300003000300309111133330533335=++++----=+=2012年亚太小学奥林匹克第一回合图中ABCD 为平行四边形。
四条边的中点分别为P ,Q ,R 和S 。
已知阴影部分的面积为220cm ,请问平行四边形ABCD 的面积为多少2cm ?D【分析与解】几何,面积,割补。
阴影部分的面积是平行四边形ABCD 的面积的15;平行四边形ABCD 的面积是阴影部分面积的5倍; 平行四边形ABCD 的面积为2205100cm ⨯=。
2012年亚太小学奥林匹克第一回合小珍将以下正整数中的所有数字相加,得到一个新的数1n 。
201233333⨯⨯⨯⨯个 然后,她将1n 中的所有数字相加,得到另一个新的数2n 。
她不断重复以上操作,直到加出一个个位数为止。
试求该个位数。
【分析与解】 数论,整除。
2012320103333393333⨯⨯⨯⨯=⨯⨯⨯⨯⨯个个是9的倍数; 如果一个数是9的倍数,那么这个数的数字之和也是9的倍数; 故1n ,2n ,…都是9的倍数;0~9中,只有0和9是9的倍数;而一个非零自然数,将其数字相加,数字之和不可能是0; 故该个位数是9。
【第4题】如图所示大中小三个圆,小圆的圆周经过中圆的圆心,中圆的圆周又经过大圆的圆心。
2009年APMO亚太区数学奥林匹克真题(中文版)

ù ø =Øbç(APMO)¬ˇtæªˇvÈ:2009Ä3~10nªˇËõ:2Û×ç òÃ×ç·<9á:…tæíqñcªâAPMOëjæ¦êÓ Êëj£ êÓ‡,~§¢Ð#LSA,«w u%âæ˜f]vÈÌ…:lûüv(9:30am∼1:30pm)ÎTÇÕ,Á Ìà.H C H°zŸ Á .)J^£¯(Á)^£l .â©°t»> .)UàlÂÂ…t»uüæ,©æÅ}þ}½æø úŸÊ.$,í£õbªWà-ÍT:²ø_ŸÊ.$,íb r,Ó¥¥_b,Í(Ê.$,Ÿ,Å—2r2=abíøú£õb a,b.c qøÇá.$,É ø_£õb r,/ÍT k2−1Ÿ()ƒk2_£õb(¥< b.øìbóæ) …p.$,æÊø_b/¤b.Ĭkr.Problem1.Consider the following operation on positive real numbers written on a blackboard:Choose a number r written on the blackboard,erase that number,and then write a pair of positive real numbers a and b satisfying the condition2r2=ab on the board.Assume that you start out with just one positive real number r on the blackboard, and apply this operation k2−1times to end up with k2positive real numbers,not necessarily distinct.Show that there exists a number on the board which does not exceed kr.½æù I a1,a2,a3,a4,a5uÅ—- j˙ íõb:úk k=1,2,3,4,5,a1k2+1+a2k2+2+a3k2+3+a4k2+4+a5k2+5=1k2.t°a137+a238+a339+a440+a5415M (J| }b[5)Problem2.Let a1,a2,a3,a4,a5be real numbers satisfying the following equations:a1 k2+1+a2k2+2+a3k2+3+a4k2+4+a5k2+5=1k2for k=1,2,3,4,5.Find the value of a137+a238+a339+a440+a541.(Express the value in a single fraction.)½æú ÊøÃÞ,#ì˛¤.ó>/Õ×íúÆΓ1,Γ2,Γ3.úkʤÃÞ,/Êú_ÆÕ¶íLøõP,Z¨ý_õA1,B1,A2,B2,A3,B3à-:úk©_i=1,2,3, A i,B iÑÆΓi,íóæsõ/U)ò(P A i£P B iîDΓió~ %â¤Z¨j ,à‹ú(A1B1,A2B2,A3B3uõ†˚PÑÔæõ t…à‹Ôæõæʆ¤ÃÞ,í©ø_ÔæõîrÊ°ø_Æ,Problem3.Let three circlesΓ1,Γ2,Γ3,which are non-overlapping and mutually external,be given in the plane.For each point P in the plane,outside the three circles,construct six points A1,B1,A2,B2,A3,B3as follows:For each i=1,2,3, A i,B i are distinct points on the circleΓi such that the lines P A i and P B i are both tangents toΓi.Call the point P exceptional if,from the construction,three lines A1B1,A2B2,A3B3are concurrent.Show that every exceptional point of the plane, if exists,lies on the same circle.½æû t…úL<£cb k,æÊø_ Übí Ïba1 b1,a2b2,···,a kb kw2ú©_i=1,2,...,k,a i D b i uÔí£cb,1/£cb a1,b1,a2,b2,...,a k,b k îóæProblem4.Prove that for any positive integer k,there exists an arithmetic se-quencea1 b1,a2b2,···,a kb kof rational numbers,where a i,b i are relatively prime positive integers for each i= 1,2,...,k,such that the positive integers a1,b1,a2,b2,...,a k,b k are all distinct.½æü s_œÂA‘¯˝’D‘¯¬’u°ÿ ø0š,HÂ*–õ“Ù®”|ꃮ õ”½i” s_œÂA·ªJÍT j²b,7/éšäOÎJ-íd† :*–õ–,©• t¼‘¯˝’ÿHj²béšä%˝ ;*–õ–,©•r t¼‘¯¬’ÿHj²béšä%¬ ,¤T ,r uÔí£cb çs_A°vªJ Hj²b v,šäÿ. ,./%‡• c qËÞuÃí,7/šäªJ•%LSø_j² øÇášä*“Ù®”Çá, O“½i”íj²|ê ~½,úk¨<bú( ,r), .Óٮ”D“½i”ó×Ö±,šäøì?•ƒ“½i”?rry and Rob are two robots travelling in one car from Argovia to Zillis.Both robots have control over the steering and steer according to the following algorithm:Larry makes a90◦left turn after every kilometer driving from start; Rob makes a90◦right turn after every r kilometer driving from start,where and r are relatively prime positive integers.In the event of both turns occurring simultaneously,the car will keep going without changing direction.Assume that the ground isflat and the car move in any direction.Let the car start from Argovia facing towards Zillis.For which choices of the pair ( ,r)is the car guaranteed to reach Zillis,regardless of how far it is from Argovia?。
2013年第25届APMO亚太区数学奥林匹克真题(英文版)

XXV Asian Pacific Mathematics OlympiadTime allowed:4hours Each problem if worth7points Problem1.Let ABC be an acute triangle with altitudes AD,BE and CF,and let O be the center of its circumcircle.Show that the segments OA,OF,OB,OD,OC,OE dissect the triangle ABC into three pairs of triangles that have equal areas.Problem2.Determine all positive integers n for whichn2+1[√n]2+2is an integer.Here[r]denotes the greatest integer less than or equal to r.Problem3.For2k real numbers a1,a2,...,a k,b1,b2,...,b k define the sequence of numbers X n byX n=ki=1[a i n+b i](n=1,2,...).If the sequence X n forms an arithmetic progression,show that ki=1a i must be aninteger.Here[r]denotes the greatest integer less than or equal to r.Problem4.Let a and b be positive integers,and let A and B befinite sets of integers satisfying:(i)A and B are disjoint;(ii)if an integer i belongs either to A or to B,then i+a belongs to A or i−b belongs to B.Prove that a|A|=b|B|.(Here|X|denotes the number of elements in the set X.) Problem5.Let ABCD be a quadrilateral inscribed in a circleω,and let P be a point on the extension of AC such that P B and P D are tangent toω.The tangent at C intersects P D at Q and the line AD at R.Let E be the second point of intersection between AQ andω.Prove that B,E,R are collinear.。
【五年级】亚太区「奥数之星」数学奥林匹克邀请赛

题目及答题纸题目及答题纸Problem & Answer Paper时限时限Time allowed:90分钟分钟minutes 考生须知考生须知Instructions to Candidates:1.每位考生将获「题目及答题纸」乙份。
每位考生将获「题目及答题纸」乙份。
ONE “problem and answer paper” will be given for each candidate.2.本卷共120分,第1至10题(每题7分),第11至15题(每题10分)。
本卷共120分,第1至10题(每题7分),第11至15题(每题10分)。
This paper contains 120 marks, questions 1-10 (7 marks each), questions 11-15 (10 marks each).3.比赛期间,不得使用计算工具或手形。
比赛期间,不得使用计算工具或手形。
NO calculation instruments or hand sign during the contest.4.请将答案写在本卷上。
请将答案写在本卷上。
Write down all answers on this paper.5.比赛完毕时,所有「题目及答题纸」会被收回。
比赛完毕时,所有「题目及答题纸」会被收回。
ALL “problem and answer papers” will be collected at the end of the contest.6.本卷中所有附图不一定依比例绘成。
本卷中所有附图不一定依比例绘成。
The diagrams in this paper are NOT necessarily drawn in scale.若计算结果是分数,请化至最简,并确保为真分数或带分数,或将计算结果写成小数。
若计算结果是分数,请化至最简,并确保为真分数或带分数,或将计算结果写成小数。
历年各杯赛奥数详解

历年各杯赛奥数详解奥数(Olympiad Mathematics)是指数学界的奥林匹克竞赛,是世界数学界的一次重要盛事。
历年来,各个国家和地区都举办了各种各样的奥数比赛,旨在鼓励和培养年轻学生的数学兴趣和创新能力。
本文将对历年各杯赛奥数进行详细的介绍和分析,以帮助读者深入了解奥数竞赛的发展历程和重要性。
一、国际奥数比赛1. 国际数学奥林匹克竞赛(IMO)国际数学奥林匹克竞赛是世界上最著名的奥数比赛之一,始于1959年,每年一届。
该比赛是针对全球高中学生组织的,其题目难度非常高,需要参赛学生具备深厚的数学知识和解题思维能力。
2. 国际信息学奥林匹克竞赛(IOI)国际信息学奥林匹克竞赛是专门针对计算机科学领域的奥数比赛,旨在培养学生的算法和编程能力。
参赛学生需要通过编程解决一系列复杂的问题,这对于提高他们的逻辑思维和问题解决能力非常有帮助。
3. 国际物理奥林匹克竞赛(IPhO)国际物理奥林匹克竞赛是对物理学方向的奥数比赛,要求参赛学生具备扎实的物理理论知识和实践操作能力。
比赛题目通常涉及到独特的物理实验和复杂的理论计算,对学生的创新思维和实践操作能力提出了很高的要求。
二、地区性奥数比赛1. 亚洲太平洋地区奥林匹克竞赛(APMO)亚洲太平洋地区奥数比赛是针对亚洲国家和太平洋地区的高中学生组织的,与IMO类似,也是一项世界级的奥数竞赛。
该比赛涉及到的数学领域更广泛,要求参赛学生具备更全面的数学知识和解题能力。
2. 欧洲女子数学奥林匹克竞赛(EGMO)欧洲女子数学奥林匹克竞赛以鼓励女性学生参与奥数竞赛为目标,是欧洲地区专门举办的数学比赛。
与其他奥数竞赛不同的是,EGMO 注重鼓励女性学生在数学领域的发展,并提供平等机会来展示她们的数学才能。
三、国内奥数比赛1. 全国青少年数学天才大赛全国青少年数学天才大赛是中国最为著名和权威的奥数比赛之一,吸引了全国各地的优秀数学学生参赛。
该比赛注重考察学生的综合数学素养和解题能力,题目种类多样,既有应用题,也有纯理论题,对学生的数学基础和思维能力提出了较高要求。
2013AIMO台湾大学总决赛4年级试题

四年級 / 小學四年級 四年级 / 小学四年级
問題簿 问题簿
Grade 4 / Primary 4 Question Book
2)
3)
4)
5)
6)
7) 求 1494 1494 494 494 的值。 求 1494 1494 494 494 的值。 Evaluate 1494 1494 494 494 . 8) 甲和乙各有若干塊橡皮擦,如果甲用完 14 塊橡皮擦,乙用完 17 塊橡皮擦,則二人剩下相同數目 的橡皮擦未用;如果老師給了甲 5 塊橡皮擦,要使甲乙二人剩下相同數目的橡皮擦未用,老師應 該要給乙多少塊橡皮擦? 甲和乙各有若干块橡皮擦,如果甲用完 14 块橡皮擦,乙用完 17 块橡皮擦,则二人剩下相同数目 的橡皮擦未用;如果老师给了甲 5 块橡皮擦,要使甲乙二人剩下相同数目的橡皮擦未用,老师应 该要给乙多少块橡皮擦? Student A and student B have some erasers. If student A uses 14 erasers and student B uses 17 erasers, then they will have the same number of erasers. If a teacher gives 5 erasers to student A, how many erasers should the teacher give to student B so that the two students will have the same number of erasers? 某一個兩位數,十位與個位數字和為 10,若將十位與個位的數字對調後,新數比原數大 36,求 原來的兩位數。 某一个两位数,十位与个位数字和为 10,若将十位与个位的数字对调后,新数比原数大 36,求
2024年西班牙数学奥林匹克竞赛数学试卷(含答案解析)

2024年竞赛数学试卷西班牙数学奥林匹克一、解答题1、2024个不同的素数pp1, pp2,⋯ , pp2024满足条件: pp1+pp2+⋯+pp1012=pp1013+pp1014+⋯+ pp2024设AA=pp1pp2⋯pp1012,BB=pp1013pp1014⋯pp2024⋅求证: |AA−BB|≥4.2、给定正整数nn ,实数xx1, xx2,⋯ ,xx nn>1满足xx1xx2⋯xx nn=nn+1.求证:(112(xx1−1)+1)(122(xx2−1)+ 1)⋯(1nn2(xx nn−1)+1)≥nn+1,并说明等号何时成立.3、设△AABBAA为不等边三角形,PP为三角形内部一点,满足∠PPBBAA=∠PPAAAA. 直线PPBB和∠BBAAAA的内角平分线交于点QQ,直线PPAA和∠BBAAAA的外角平分线交于点RR.点SS满足AASS∥AAQQ,BBSS∥AARR,求证:QQ,RR,SS三点共线.4、实数aa,bb,cc,dd满足aabbccdd=1,aa+1aa+bb+1bb+cc+1cc+dd+1dd=0求证:aabb,aacc,aadd中至少一个等于−1.5、给定平面上的两点pp1=(xx1,yy1),pp2=(xx2,yy2),用RR(pp1,pp2)表示边与坐标轴平行、且以pp1和pp2为对角顶点的矩形,即�(xx,yy)∈RR2|mmmm nn{xx1,xx2}≤xx≤mmaaxx{xx1,xx2},mmmm nn{yy1,yy2}≤yy≤mmaaxx{yy1,yy2}�.若对所有的点集SS⊂RR2,且|SS|=2024,都存在两点pp1,pp2∈SS,使得|SS∩RR(pp1,pp2)|≥,求kk的最大可能值.6、设aa,bb,nn为正整数,满足bbmm整除aann−aa+1,记αα=aa bb.求证:[αα],[2αα],…,[(nn−1)αα]除以nn的余数是1,2,⋯ ,nn−1的一个排列.1 、【答案】 见解析;【解析】 证明:首先注意到2∉{pp ii }1≤ii ≤2024,若不然易证明等式两侧的奇偶性不同,矛盾!因此本题中的pp 1, pp 2,⋯, pp 2024都是奇数,因此 pp ii ≡±1(mmmmdd 4),mm =1,2...2024 设AA 中有xx 个质数是mmmmdd 4余1的,则有 (1012−xx )个数是余−1的;同理设BB 中有yy 个质数是mmmmddpp 余1的,则有 (1012−yy )个数是余−1的,于是我们有 xx −(1012−xx )≡yy −(1012−yy )(mmmmdd 4) 这意味着xx ≡yy (mmmmdd 2),那么 ≡(−1)1012−xx ≡(−1)1012−yy ≡BB (mmmmdd 4)注意到AA ≡0(mmmmddpp 1),而BB ≡0(mmmmddpp 1)不成立,因此AA ≠BB ,进而|AA −BB |≥4,得证.【标注】 2 、【答案】 见解析;【解析】 证明:注意到1+1kk 2(xx kk −1)−(kk+1)2kk 2xx kk =kk 2xx kk (xx kk −1)+xx kk −(xx kk −1)(kk+1)kk 2xx kk (xx kk −1) =kk 2xx kk 2−kk 2xx kk +xx kk −(xx kk −1)(kk+1)2kk 2xx kk (xx kk −1) kk 2xx kk 2−kk 2xx kk +xx kk −xx kk (kk+1)2+(kk+1)2kk 2xx kk (xx kk −1) =kk 2xx kk 2−kk 2xx kk +xx kk −kk 2xx kk −2kkxx kk −xx kk +(kk+1)2kk 2xx kk (xx kk −1) =kk 2xx kk 2−2kkxx kk (kk+1)+(kk+1)2kk 2xx kk (xx kk −1) =(kkxx kk −kk−1)2kk 2xx kk (xx kk −1)⩾0 因此1+1kk 2(xx kk −1)≥(kk+1)2kk 2xx kk, 累乘可得��1+1kk 2(xx kk −1)�nn kk=1≥(nn+1)2xx 1xx 2⋯xx nn =nn +1,等号成立当且仅当xx kk =kk+1kk 时取得. 【标注】 3 、【答案】 见解析;【解析】 证明:我们记AARR ,BBPP 交于点DD ,AAQQ ,BBSS 交于点EE .由于∠AABBPP =∠AAAAPP ,∠BBAADD =∠AAAA ,因 ΔAABBDD ∼ΔAAAARR ,则 AAAA AAAA =AAAA AAAA由角平分线的性质,易知AAAA AAAA=AABB AABB.因此AAAA AAAA=AABB AABB,这意味着AAEE,BBDD,SSRR三线共点,即QQ,RR,SS三点共线.得证.【标注】4 、【答案】见解析;【解析】证明:由题意可得0=aa+bb+cc+dd+aabbcc+aabbdd+aaccdd+bbccdd=aa+bb+cc+dd+aabb(cc+dd)+ccdd(aa+bb)=(aa+ bb)(ccdd+1)+(cc+dd)(aabb+1)=(aa+bb)(ccdd+1)+(cc+dd)(aabb+aabbccdd)=(aa+bb)(ccdd+1)+ aabb(cc+dd)(ccdd+1)=(ccdd+1)(aa+bb+aabbcc+aabbdd=(ccdd+1)[aa(1+bbcc)+bb(1+aadd)]=(ccdd+ 1)[aa(1+bbcc)+bb(aabbccdd+aadd)]=(ccdd+1)[aa(1+bbcc)+aabbdd(1+bbcc)]=aa(ccdd+1)(bbcc+1)(1+ bbdd)这意味着在ccdd,bbcc,bbdd中至少有一个是−1,结合aabbccdd=1可知在aabb,aacc,aadd中至少有一个是−1,得证.【标注】5 、【答案】406;【解析】证明:设在点集SS中,点PP是纵坐标最大的点,QQ是横坐标最大的点,R是纵坐标最小的点,SS是横坐标最小的点.我们将RR(XX,YY)简化为(XX,YY).考虑(PP,QQ),(QQ,RR),(RR,SS),(SS,PP)这四个矩形,设它们包含的点的个数分别是aa,bb,cc,dd.若它们之间有相互重叠的部分,由极端值原理可知mmaaxx{aa,bb,cc,dd}≥aa+bb+cc+dd4≥20244=506若它们之间没有重叠部分,则在整个SS中,除了上述四个小矩形之外,中间还有一个小矩形,设其内部有tt个点,此时(PP,RR)至少含有tt+2个点,注意到此时aa+bb+cc+dd+tt−4=2024,则由极端值原理可知{aa,bb,cc,dd,tt+2}≥aa+bb+cc+dd+tt+25=406,,这说明至少有一个区域含有406个点,即kk mmaaxx=406.下面我们给出kk=406时的一个构造,如图所示.四条线段上各有406个点,中间的环上有404个点【标注】6 、【答案】见解析;【解析】证明:当nn=1时命题显然成立,只需考虑nn≥2时的情况即可.但注意到要证明该命题成立,只需同时证明|ααkk|≠0(mmmmddnn)和[ααmm]≠[αααα](mmmmddnn)即可,下分别证之.(1) [ααkk]≠0(mmmmddnn)反设存在kk∈{1,2,nn−1}使得[ααkk]=0(mmmmddnn),记aakk≡tt(mmmmddbb)⟹nnbb|aakk−tt,但由题意可得aa(nn−1)≡−1(mmmmddbb),我们有bbnn|(nn−1)tt+kk,然而(nn−1)tt+kk≤(nn−1)(bb−1)+nn−1= (nn−1)bb<nnbb矛盾!(2) [aamm]≠[aaαα](mmmmddnn)我们反设假设存在i,αα∈{1,2,...nn−1},mm≠αα,使得[ααmm]≡[αααα](mmmmddnn).不妨记aamm≡pp(mmmmddbb)和aaαα≡qq(mmmmddbb),从而bbnn|aamm−pp,bbnn|aaαα−qq,因此aa(mm−αα)=pp−qq(mmmmddbbnn)注意到aa(nn−1)≡−1(mmmmddbbnn),因此bbnn|(nn−1)(pp−qq)−(mm−αα),然而(nn−1)(pp−qq)−(mm−αα)|≤|(nn−1)(pp−qq)|+|mm−αα|≤(nn−1)(bb−1)+(nn−2)<bb因此只能是(nn−1)(pp−qq)−(mm−αα)=0,但|mm−αα|≤nn−2因此两侧关于nn−1不同余,矛盾【标注】。
2013年AMC 10B竞赛真题及答案(英文版)

2013年美国数学竞赛AMC10B真题What is ?Problem 2Mr. Green measures his rectangular garden by walking two of the sides and finding that it is steps by steps. Each of Mr. Green's steps is feet long. Mr. Green expects a half a pound of potatoes persquare foot from his garden. How many pounds of potatoes does Mr. Green expect from his garden?Problem 3On a particular January day, the high temperature in Lincoln, Nebraska, was degrees higher than the low temperature, and the average of the high and the low temperatures was . In degrees, whatwas the low temperature in Lincoln that day?Problem 4When counting from to , is the number counted. When counting backwardsfrom to , is the number counted. What is ?Problem 5Positive integers and are each less than . What is the smallest possible value for ?The average age of 33 fifth-graders is 11. The average age of 55 of their parents is 33. What is the average age of all of these parents and fifth-graders?Problem 7Six points are equally spaced around a circle of radius 1. Three of these points are the vertices of atriangle that is neither equilateral nor isosceles. What is the area of this triangle?Problem 8Ray's car averages 40 miles per gallon of gasoline, and Tom's car averages 10 miles per gallon of gasoline. Ray and Tom each drive the same number of miles. What is the cars' combined rate of milesper gallon of gasoline?Problem 9Three positive integers are each greater than , have a product of , and are pairwise relativelyprime. What is their sum?Problem 10A basketball team's players were successful on 50% of their two-point shots and 40% of their three-point shots, which resulted in 54 points. They attempted 50% more two-point shots than three-point shots. How many three-point shots did they attempt?Real numbers and satisfy the equation . What is ?Problem 12Let be the set of sides and diagonals of a regular pentagon. A pair of elements of are selected atrandom without replacement. What is the probability that the two chosen segments have the samelength?Problem 13Jo and Blair take turns counting from to one more than the last number said by the other person. Jostarts by saying "", so Blair follows by saying "" . Jo then says "" , and so on. What is the53rd number said?Problem 14Define . Which of the following describes the set of points forwhich ?Problem 15A wire is cut into two pieces, one of length and the other of length . The piece of length is bent to form an equilateral triangle, and the piece of length is bent to form a regular hexagon. The triangle and the hexagon have equal area. What is ?In triangle , medians and intersect at , , , and . What isthe area of ?Problem 17Alex has red tokens and blue tokens. There is a booth where Alex can give two red tokens andreceive in return a silver token and a blue token, and another booth where Alex can give three blue tokens and receive in return a silver token and a red token. Alex continues to exchange tokens until nomore exchanges are possible. How many silver tokens will Alex have at the end?Problem 18The number has the property that its units digit is the sum of its other digits, thatis . How many integers less than but greater than share this property?Problem 19The real numbers form an arithmetic sequence with . Thequadratic has exactly one root. What is this root?Problem 20The number is expressed in the formwhere and are positive integers and is as small aspossible. What is ?Two non-decreasing sequences of nonnegative integers have different first terms. Each sequence has the property that each term beginning with the third is the sum of the previous two terms, and the seventh term of each sequence is . What is the smallest possible value of N?Problem 22The regular octagon has its center at . Each of the vertices and the center are to beassociated with one of the digits through , with each digit used once, in such a way that the sums ofthe numbers on the lines , , , and are all equal. In how many ways can this bedone?In triangle , , , and . Distinct points , , and lie onsegments , , and , respectively, such that , , and . Thelength of segment can be written as , where and are relatively prime positive integers.What is ?Problem 24A positive integer is nice if there is a positive integer with exactly four positive divisors (including and ) such that the sum of the four divisors is equal to . How many numbers in theset are nice?Bernardo chooses a three-digit positive integer and writes both its base-5 and base-6 representations on a blackboard. Later LeRoy sees the two numbers Bernardo has written. Treating the two numbers as base-10 integers, he adds them to obtain an integer . For example, if ,Bernardo writes the numbers and , and LeRoy obtains the sum . For how many choices of are the two rightmost digits of , in order, the same as those of ?Answer Key1. C2. A3. C4. D5. B6. C7. B8. B9. D10.C11.B12.B13.E14.E15.B16.B17.E18.D19.D20.B21.C22.C23.B24.A25.E。
2014年第26届APMO亚太区数学奥林匹克答案详解(英文版)

ai to be a positive integer among whose digits the number 2 appears exactly k + i − 2 times and the number 1 appears exactly 2k+i−1 − 2(k + i − 2) times, and nothing else. Then, we have S(ai) = 2k+i−1 and P (ai) = 2k+i−2 for each i, 2 ≤ i ≤ n. Then, we let a1 be a positive integer among whose digits the number 2 appears exactly k + n − 1 times and the number 1 appears exactly 2k − 2(k + n − 1) times, and nothing else. Then, we see that a1 satisfies S(a1) = 2k and P (a1) = 2k+n−1. Such a choice of a1 is possible if we take k to be large enough to satisfy 2k > 2(k + n − 1) and we see that the numbers a1, . . . , an chosen this way satisfy the given requirements.
2014年第25届亚太杯上海初赛试题解析

亚太小学数学奥林匹克(上海赛区初赛 五年级 A卷 2小时 总分150分)1. 22221+2+3++2014 的运算结果的奇偶性为______。
(填“奇数”或“偶数”)【答案】奇数;【解析】奇偶分析;1,2,3,……,2014中有1007个奇数;奇数的平方仍是奇数,偶数的平方仍是偶数,所以,原式中的和为奇数。
2. 计算:2014201420132015×−×=__________。
【答案】1【解析】平方差公式2222014(20141)(20141)2014(20141)1=−−×+=−−=原式3. 若六位数222 能被25整除,这样的六位数中,最大的是________。
【答案】922275 【解析】数的整除能被25整除的,末两位数是且只能是00,25,50,75中的一种。
4. 设a b Δ表示a 的2倍减去b 的3倍,也即23a b a b Δ=−,已知(1)20x ΔΔ=,则x =___________。
【答案】3【解析】自定义运算(1)2(23)22(23)3241203x x x x x ΔΔ=−Δ=−−×=−= =5. 若149ab cd +=,则a b c d +++=__________。
【答案】23【解析】进位问题 + 1 4 9a bc d如图,个位数,b d 不可能进位,所以:92314b d a b c d a c +=⎫⇒+++=⎬+=⎭6. 已知有一串数1223334444 、、、、、、、、、、。
这串数中的第40个数是_____。
【答案】9【解析】等差数列,循环问题 当8n =时,112(1)36402n n n +++=+=< 当9n =时,112(1)45402n n n +++=+=>7. 已知23208a b +=,且a b 、均为质数,则ab 的值为________。
【答案】202【解析】奇偶性质232083210122a b b b a a b ⎫+=⎫⇒⎬⎪⇒=⇒=⎬⎭⎪⎭是偶数是偶数是质数,是唯一偶质数1012202ab ∴=×=8. 数学小组中有3名男生和4名女生,现要从这7人中选1名男生1和名女生组成代表队,共有________种组队方式。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
Problem 1. Let ABC be an acute triangle with altitudes AD, BE and CF , and let O be the center of its circumcircle. Show that the segments OA, OF, OB, OD, OC, OE dissect the triangle ABC into three pairs of triangles that have equal areas. Solution. Let M and N be midpoints of sides BC and AC, respectively. Notice that 1 ∠BOC = ∠EAB, ∠OM C = 90◦ = ∠AEB, so triangles OM C and AEB are ∠M OC = 2 OA = OC . For triangles ON A and BDA we also have ON = BA . Then similar and we get OM AE AB BD OM ON = BD or BD · OM = AE · ON. AE Denote by S (Φ) the area of the figure Φ. So, we see that S (OBD) = 1 BD · OM = 2 1 AE · ON = S (OAE ). Analogously, S (OCD) = S (OAF ) and S (OCE ) = S (OBF ). 2 Alternative solution. Let R be the circumradius of triangle ABC , and as usual write A, B, C for angles ∠CAB , ∠ABC , ∠BCA respectively, and a, b, c for sides BC , CA, AB respectively. Then the area of triangle OCD is S (OCD) = Now CD = b cos C , and
k must hold. Since this inequality holds for any from which we conclude that |A − d| < n positive integer n, we must have A = d. Since {Xn } is a sequence of integers, d must be an integer also, and thus we conclude that A is also an integer.
k
Xn =
i=1
[ai n + bi ] (n = 1, 2, . ቤተ መጻሕፍቲ ባይዱ .).
k i=1
If the sequence Xn forms an arithmetic progression, show that Here [r] denotes the greatest integer less than or equal to r. Solution. Let us write A = k i=1 ai and B = of the following inequalities over i,
Thus, A ∪ B = A∗ ∪ B ∗ and A∗ and B ∗ have no element in common. For each finite set X of integers, let (X ) = x∈X x. Then (A) + (B ) = = = which implies a|A| = b|B |. Alternative solution. Let us construct a directed graph whose vertices are labelled by the members of A ∪ B and such that there is an edge from i to j iff j ∈ A and j = i + a or j ∈ B and j = i − b. From (ii), each vertex has out-degree ≥ 1 and, from (i), each vertex has in-degree ≤ 1. Since the sum of the out-degrees equals the sum of the in-degrees, each vertex has in-degree and out-degree equal to 1. This is only possible if the graph is the union of disjoint cycles, say G1 , G2 , . . . , Gn . Let |Ak | be the number of elements of A in Gk and |Bk | be the number of elements of B in Gk . The cycle Gk will involve increasing vertex labels by a a total of |Ak | times and decreasing them by b a total of |Bk | times. Since it is a cycle, we have a|Ak | = b|Bk |. Summing over all cycles gives the result. Problem 5. Let ABCD be a quadrilateral inscribed in a circle ω , and let P be a point on the extension of AC such that P B and P D are tangent to ω . The tangent at C intersects P D at Q and the line AD at R. Let E be the second point of intersection between AQ and ω . Prove that B, E, R are collinear. Solution. To show B, E, R are collinear, it is equivalent to show the lines AD, BE, CQ are concurrent. Let CQ intersect AD at R and BE intersect AD at R . We shall show RD/RA = R D/R A so that R = R . Since P AD is similar to P DC and P AB is similar to P BC , we have AD/DC = P A/P D = P A/P B = AB/BC . Hence, AB · DC = BC · AD. By Ptolemy’s theorem, 1 CA · DB . Similarly CA · ED = CE · AD = 1 AE · DC . AB · DC = BC · AD = 2 2 Thus DB 2DC = , (3) AB CA and DC 2ED = . (4) CA AE (A ∪ B ) (A∗ ∪ B ∗ ) = (A) − a|A| + (A∗ ) + (B ∗ ) (2)
n2 +1 √ [ n]2 +2
is an integer. Here [r]
Solution. We will show that there are no positive integers n satisfying the condition of the problem. √ Let m = [ n] and a = n − m2 . We have m ≥ 1 since n ≥ 1. From n2 +1 = (m2 + a)2 +1 ≡ (a − 2)2 + 1 (mod (m2 + 2)), it follows that the condition of the problem is equivalent to the fact that (a − 2)2 + 1 is divisible by m2 + 2. Since we have 0 < (a − 2)2 + 1 ≤ max{22 , (2m − 2)2 } + 1 ≤ 4m2 + 1 < 4(m2 + 2),
k i=1 bi .
ai must be an integer.
Summing the corresponding terms
ai n + bi − 1 < [ai n + bi ] ≤ ai n + bi , we obtain An + B − k < Xn < An + B. Now suppose that {Xn } is an arithmetic progression with the common difference d, then we have nd = Xn+1 − X1 and A + B − k < X1 ≤ A + B Combining with the inequalities obtained above, we get A(n + 1) + B − k < nd + X1 < A(n + 1) + B, or An − k ≤ An + (A + B − X1 ) − k < nd < An + (A + B − X1 ) < An + k,
