组合数学试题2012
组合数学期末试题

组合数学期末试题期末试卷2012—2013学年第二学期课程:组合数学专业:数学与应用数学年级:2010本试卷共2页满分:100分考试时间:120分钟考试方式:闭卷一、填空题(本大题共8小题,每小题2分,共16分)1、将5个苹果分给3个小孩,有_______种不同的分法.2、多项式()4012324x x x x +++中项22012x x x ??的系数是 .3、22件产品中有2件次品,任取3件,恰有一件次品方式数为________.4、Fibbonacci 数F(9)= .5、6()x y +所有项的系数和是________.6、含3个变元,,x y z 的一个对称多项式包含9个项,其中4项包含x ,2项包含xyz ,1项包含常数项,求包含xy 的项有个.7、在{1,2,3,4,5,6}全排列中,使得只有偶数在原来位置的排列方式数为 .8、把某英语兴趣班分成两个小组,甲组有2名男同学,5名女同学;乙组有3名男同学,6名女同学,从甲乙两组均选出3名同学来比赛,则选出的6人中恰有1名男同学的方式数 .二、单项选择题(本大题共8小题,每小题3分,共24分)9、在一次聚会上有15位男士和20位女士,则形成15对男女一共有多少种方式数()A 、20!5!B 、20!15!C 、2015D 、152010、某年级的课外学科小组分为数学、语文二个小组,参加数学小组的有23人,参加语文小组的有27人;同时参加数学、语文两个小组的有7人。
这个年级参加课外学科小组人数()。
B 、57C 、43D 、1111、组合式???? ??50120与下列哪个式子相等?()A 、???? ??60120B 、???? ??50119+???? ??49119C 、512???? ??49120D 、???? ??4911912、从1至1000的整数中,有多少个整数能被5整除但不能被6整除?()A 、167B 、200C 、166D 、3313、商店有六种饮料供选择,若小明每天至少和一种饮料(喝过的不再选择),5天里把全部饮料都喝过,则有多少种不同的安排?()A 、9B 、16C 、90D 、180014、...0110p q p q p q r r r +++= ??? ??? ???-????????()min{,}r p q ≤。
2012组合教育密押三套卷全国卷答案

2 3 1 3 3 3 , (VS − ABC ) max = . 故选 D. × = ×1 = 3 3 3 3 2 12.A【解析】依题意,直线 ax + by = 1 与线段 AB 有一个公共点,
且此时 OH = 则 ( a − 1)( 2a + b − 1) - 0 , a , b 所满足的可行域如图所示,
1 1 AB ,因为 DC ∥ AB ,所以 MN ∥DC . 2 2 所以四边形 MNCD 是平行四边形,所以 DM ∥CN . ……………………(10 分) 因为 DM ⊄ 平面 PBC , CN ⊂ 平面 PBC ,所以 DM ∥ 平面 PBC , …………………(12 分) 所以,当 M 为 AP 的中点时, MD∥ 平面 PBC . 19.(理)【解析】(1)用 A, B, C 分别表示事件甲、乙、丙面试合格. 1 1 由题意知 A, B, C 相互独立, 且 P ( A ) = ,P( B) = P( C) = . ………………… (2 分) 2 3
(
) (
) (
)
所以 b = 1 . 椭圆 C 的方程为
2
x2 + y2 = 1 . 4
………………(4 分)
(
)
(2)由△ OEF 为直角三角形.
1 e
二、填空题
2
; 7. C【解析】当 ln x > 0 时,即 x > 1 , f ( x) = 1 − ln x ,令 f ( x ) = 0 得 x = e 或 x = (舍) 当 ln x = 0 时,即 x = 1 , f ( x) = − ln x ,令 f ( x ) = 0 ,得 x = 1 ;
2012年高考数学备考之百所名校组合卷系列_专题08_解析几何

一、选择题:1. (浙江省宁波市鄞州区2012年3月高考适应性考试文科5)已知实数4,m ,9构成一个等比数列,则圆锥曲线122=+y mx 的离心率为( ) 630.A 7.B 7630.或C 765.或D 【答案】C【解析】本题主要考查圆锥曲线的定义及简单性质。
实数4,m ,9构成一个等比数列,则236m =,即6m =±;当6m =时,曲线方程2216x y +=表示焦点在x 轴上的椭圆,根据1,a b c ===则c e a ===。
当6m =-时,曲线方程2216x y -=表示焦点在y 轴上的双曲线,根据1,a b c ===则1c e a === C. 2. (山东实验中学2012届高三第一次诊断性考试理12)点P在双曲线上•,是这条双曲线的两个焦点,,且的三条边长成等差数列,则此双曲线的离心率是 (A) .2 (B) .3(C) .4(D) .5【答案】D【解析】设|PF 2|,|PF 1|,|F 1F 2|成等差数列,且分别设为m-d,m,m+d,则由双曲线定义和勾股定理可知:m-(m-d)=2a,m+d=2c, (m-d)2+m 2=(m+d)2,解得m=4d=8a,5252dce da ∴===故选项为D3. (吉林省长春市2012年3月高中毕业班第二次调研测试理科11)以O 为中心,1F ,2F 为两个焦点的椭圆上存在一点M ,满足1222MF MO MF ==,则该椭圆的离心率为A.3B.23C.3D.5【答案】C【解析】过M 作x 轴的垂线,交x 轴于N 点,则N 点坐标为(,0)2c,并设12222MF MO MF t ===,根据勾股定理可知,22221122MF NFMF NF -=-,得到2c t =,而32ta =,则3c e a ==.4.(北京市东城区2012届高三上学期期末考试文6)在平面直角坐标系内,若曲线C :22224540x y ax ay a ++-+-=上所有的点均在第二象限内,则实数a 的取值范围为(A )(),2-∞- (B )(),1-∞- (C )()1,+∞ (D )()2,+∞【答案】D【解析】曲线C :22224540x y ax ay a ++-+-=,即22()(2)4x a y a ++-=表示以(,2)a a -为圆心,2为半径的圆,当2a -<-且20a >,即2a >时,曲线C 上所有的点均在第二象限内。
高考数学试题解析专题11 排列组合、二项式定理(教师版) 理

2012年高考试题分项版解析数学(理科)专题11 排列组合、二项式定理(教师版)一、选择题:1.(2012年高考新课标全国卷理科2)将2名教师,4名学生分成2个小组,分别安排到甲、乙两地参加社会实践活动,每个小组由1名教师和2名学生组成,不同的安排方案共有()()A12种()B10种()C9种()D8种【答案】A【解析】甲地由1名教师和2名学生:122412C C=种.2. (2012年高考北京卷理科6)从0,2中选一个数字.从1.3.5中选两个数字,组成无重复数字的三位数.其中奇数的个数为( )A. 24B. 18C. 12D. 63.(2012年高考浙江卷理科6)若从1,2,2,…,9这9个整数中同时取4个不同的数,其和为偶数,则不同的取法共有()A.60种 B.63种 C.65种 D.66种4.(2012年高考山东卷理科11)现有16张不同的卡片,其中红色、黄色、蓝色、绿色卡片各4张,从中任取3张,要求这些卡片不能是同一种颜色,且红色卡片至多1张,不同取法的种数为()(A )232 (B)252 (C)472 (D)4845. (2012年高考辽宁卷理科5)一排9个座位坐了3个三口之家,若每家人坐在一起,则不同的坐法种数为( )(A)3×3! (B) 3×(3!)3(C)(3!)4(D) 9! 【答案】C【解析】此排列可分两步进行,先把三个家庭分别排列,每个家庭有3!种排法,三个家庭共有33!3!3!(3!)⨯⨯=种排法;再把三个家庭进行全排列有3!种排法。
因此不同的坐法种数为4(3!),答案为C【考点定位】本题主要考查分步计数原理,以及分析问题、解决问题的能力,属于中档题。
6.(2012年高考天津卷理科5)在251(2)x x-的二项展开式中,x 的系数为( ) (A )10 (B)-10 (C)40 (D)-407.(2012年高考安徽卷理科7)2521(2)(1)x x+-的展开式的常数项是( ) ()A 3- ()B 2- ()C 2 ()D 3 【答案】D【解析】第一个因式取2x ,第二个因式取21x得:1451(1)5C ⨯-= 第一个因式取2,第二个因式取5(1)-得:52(1)2⨯-=- 展开式的常数项是5(2)3+-=.8.(2012年高考安徽卷理科10)6位同学在毕业聚会活动中进行纪念品的交换,任意两位同学之间最多交换一次,进行交换的两位同学互赠一份纪念品,已知6位同学之间共进行了13次交换,则收到4份纪念品的同学人数为( )()A 1或3 ()B 1或4 ()C 2或3 ()D 2或4 【答案】D【解析】261315132C -=-=①设仅有甲与乙,丙没交换纪念品,则收到4份纪念品的同学人数为2人 ②设仅有甲与乙,丙与丁没交换纪念品,则收到4份纪念品的同学人数为4人. 9. (2012年高考湖北卷理科5)设a ∈Z ,且0≤a ≤13,若512012+a 能被13整除,则a=( )A.0B.1C.11D.1210. (2012年高考陕西卷理科8)两人进行乒乓球比赛,先赢3局者获胜,决出胜负为止,则所有可能出现的情形(各人输赢局次的不同视为不同情形)共有( ) (A ) 10种 (B )15种 (C ) 20种 (D ) 30种11.(2012年高考四川卷理科1)7(1)x +的展开式中2x 的系数是( ) A 、42 B 、35 C 、28 D 、21 【答案】D【解析】二项式7)1(x +展开式的通项公式为1+k T =k k x C 7,令k=2,则2273x C T 、=21C x 272=∴的系数为.【考点定位】高考二项展开式问题题型难度不大,要得到这部分分值,首先需要熟练掌握二项展开式的通项公式,其次需要强化考生的计算能力.12. (2012年高考四川卷理科11)方程22ay b x c =+中的,,{3,2,0,1,2,3}a b c ∈--,且,,a b c 互不相同,在所有这些方程所表示的曲线中,不同的抛物线共有( )A 、60条B 、62条C 、71条D 、80条13.(2012年高考全国卷理科11)将字母a,a,b,b,c,c,排成三行两列,要求每行的字母互不相同,每列的字母也互不相同,则不同的排列方法共有( ) (A )12种(B )18种(C )24种(D )36种14. (2012年高考重庆卷理科4)82x x 的展开式中常数项为( )A.1635 B.835 C.435 D.105 【答案】B 【解析】1,2x x取得次数为1:1(4:4),展开式中常数项为448135()28C ⨯=. 二、填空题:1. (2012年高考广东卷理科10)261()x x+的展开式中3x 的系数为______.(用数字作答)2. (2012年高考福建卷理科11)4)(x a +的展开式中3x 的系数等于8,则实数=a _________.【答案】2【解析】4)(x a +中含3x 的一项为r rrr x aC T -+=441,令3=r ,则83434=-a C ,即2=a .【考点定位】本题考查的知识点为二项式定理的展开式,直接应用即可.3.(2012年高考上海卷理科5)在6)2(xx -的二项展开式中,常数项等于 .4. (2012年高考湖南卷理科13) ( x x6的二项展开式中的常数项为 .(用数字作答) 【答案】-160 【解析】( 2x x6的展开式项公式是663166C (2(C 2(1)r r r r rr r r T x x x ---+==-.由题意知30,3r r -==,所以二项展开式中的常数项为33346C 2(1)160T =-=-.【考点定位】本题主要考察二项式定理,写出二项展开式的通项公式是解决这类问题的常规办法.5. (2012年高考陕西卷理科12)5()a x +展开式中2x 的系数为10, 则实数a 的值为 .6.(2012年高考全国卷理科15)若1()nx x+的展开式中第3项与第7项的二项式系数相等,则该展开式中21x的系数为 .。
同等学力-组合数学-真题库-章节分类

同等学力申硕-计算机专业-组合数学-题库一,排列与组合1.1,排列与组合1、如果五个文科生和五个理科生排成一排,共有____种不同的排法;如果要求文科生和理科生交替排成一排,则共有____种不同的排法。
(2012年真题)答:考察排列与组合(1)5个文科生和5个理科生,无限制条件,进行线排列,有10!=3628800种方案。
(2)要求文科生和理科生交替排列。
首先将5个文科生排成一排,有5!=120种方案。
然后再5个理科生排成一排,有5!=120种方案。
然后把这5个理科生插入已排好的文科生6个间隔中,选取5个间隔位的排列,为保证文理生交替,只能选择前5个间隔位或后5个间隔位,有2种方案。
于是所求为:2*5!*5!=2*120*120=28800种方案。
2、由3个a,1个b,2个c这六个元素组成的不同排列的总数是____。
(2012年真题)答:考察排列与组合所求排列总数为:6!3!1!2!=6!3!2!=60种。
3、如果四对夫妻围圆桌就座,没有任何限制条件,共有____种不同的座法;如果这四对夫妻中的四个男士和四个女士排成一排,要求男女交替,则有____种不同的排法; 如果这四对夫妻围圆桌就座, 要求夫妻相邻的座法有____种。
(2013年真题)答:考察排列与组合(1)四对夫妻共8人,无限制条件,进行圆排列,有(8-1)!=7!=5040种座法。
(2)首先将4个男士排成一排,有4!=24种方案数。
然后4个女生与男士交替排列即在男士排列后5个间隔中选取4个不同间隔位的排列,有P(5,4)= 5!/(5-4)!=5!=120种方案。
于是所求为:4!*P(5,4)=24*120=2880种方案。
(3)首先将夫妻作为一个整体,进行圆排列,有(4-1)!=3!=6种座法。
然后每对夫妻可以互相交换位置,方案数为24=16种方案。
于是所求为: 6*16=96种座法。
4、有六对夫妇坐在一个圆桌旁,其中通过转圈得到的坐法视为相同的坐法。
2012全国各地理数模拟分类汇编排列组合二项式定理

2012全国各地理数模拟分类汇编排列组合二项式定理 本套系列资料是2012年全国各地名校理科综合月考、联考、模拟试卷中的理科数学试题部分,经过精心整理而成。
具有很高的针对性和选用性,可供高考二轮选择使用1.将1,2,3,…,9这9个数字填在如图的9个空格中,要求每一行从左到右,每一列从上到下分别依次增大,当3,4固定在图中的位置时,填写空格的方法数为( A )A .6种B .12种C .18种D .24种2.将一个三位数的三个数字顺序颠倒,将所得到的数与原数相加,若和中没有一个数字是偶数,则称这个数为“奇和数”。
那么,所有的三位数中,奇和数有___100__个。
3.已知9290129(2)x a a x a x a x +=++++,则213579(3579)a a a a a ++++-2(2a + 2468468)a a a ++的值为 ( D )A .93B .103C .113D .123 4. 的展开式中合并同类项后共有( )A. 28 项B. 35项C. 42 项D. 56项5.我们将日期“20111102”即2011年11月2日称为“世界完全对称日”,那么在新千年(20010101~20991231)内的“世界完全对称日”共有( B )个 ( )A .24B .36C .720D .10006.某校高三理科实验班有5名同学报名参加甲、乙、丙三所高校的自主招生考试,每人限报一所高校.若这三所高校中每个学校都至少有1名同学报考,那么这5名同学不同的报考方法种数共有BA.144种B.150种C.196种D.256种7.“2012”含有数字0, 1, 2,且有两个数字2.则含有数字0, 1, 2,且有两个相同数字的四位数的个数为BA.18B.24C.27D.368.在某跳水运动员的一项跳水实验中,先后要完成6个动作,其中动作P只能出现在第一步或最后一步,动作Q和R实施时必须相邻,则动作顺序的编排方法共有()A. 24种B. 48种C. 96种D. 144种答案:C解析:.9.如图,在一花坛A,B,C,D四个区域种花,现有4种不同的花供选种,要求在每块里种1种花,且相邻的两块种不同的花,则不同的种法总数为A.48B.60C.72D.84答案:D解析:当A与C 同色时有4×3×3=36种不同的涂法,当A与C 不同色时有4×3×2×2=48种不同的涂法,∴共有36+48=84.10. (山东省淄博市2012年3月高三第一次模拟文科)对于各数互不相等的整数数组(i1, i2, i3…,i n)(n是不小于3的正整数),若对任意的p,q∈{1,2,3…,n},当p<q时有i p>i q,则称i p,i q 是该数组的一个“逆序”.一个数组中所有“逆序”的个数称为该数组的“逆序数”,则数组(2,4,3,1)的逆序数为 4 .。
2012年“华约”自主招生考试组合数学问题分析

2
2
2
所 以n 1 , : + 时 结论 也成 立 . 就 证 这样
明 了S n :nn 1 ( )—(- )
—
一
.
除, 但 一 a 是不被稿 , 除 则这个差必是a,2… , 中若干个数 n, ( 至少一个数 ) 之和 , 于是同① 可证结论也成立.
对 于 复 杂 的 看 似 没 有 思路 的 问题 的 深 入 思 考进 行 探 索 的 能 力.
() 1 Ⅱ 是最大. 1当 :时, I 不 因n Ⅱ (+ ) I 11 -
i =1 i =1 ≠ i ¨ ‘ “, ≠
(0 2 1= 02 即.= ¨+ 02 如果第 k 2 1— )2 1 , s s 2 1 ; 次是第 2 1 号姑娘 分 02 牌, 一张分给第 1 号姑娘 , 一张分给2 1 号姑娘 , 0l 则流动值 减少 了
试题点评 : 本题 涉 及 到 集 合 、 屉 原 理 等 知 识 . 抽 集合 问题 是 组 合 数 学 中 的一 个 重 要 内容 . 围绕 集 合 可 以研 究很 多 问题 , 中 其 分 类 讨 论 也是 组 合 中常 用 的 思 想 方 法之 一 .
要更好地备考2 1年的 自主招生 ,组合数学应该成为广大 03
Ⅱ 增大.
I =l
综上,Ⅱ 托 最大时必为 ・ 3,且p 32 +q 2 1.由于 < , 3= 03 p
I =1
21=7 ̄+x, 0361302所以Ⅱ 魁 的最大值为3 .
£ =1
试 题 点 评 : 题 全 面 考 查 了学 生 综合 利 用知 识 的 能 力 . 其 本 尤
教 论
2012年高中数学联赛试题分类排列组合

2012年高中数学联赛试题分类排列组合2012甘肃5. 11位数的手机号码,前七位数字是1390931,若余下的4 个数字只能是1、3 、5 且都至少出现1 次, 这样的手机号码有___________个;2012河北16. 马路上有编号为1,2,3,…,2011的2011只路灯,为节约用电要求关闭其中的300只灯,但不能同时关闭相邻两只,也不能关闭两端的路灯,则满足条件的关灯方法共有___3001710C _______种。
(用组合数符号表示)解答:问题等价于在1711只路灯中插入300只暗灯,所以共有3001710C 种关灯方法。
2012浙江12.如果:(1)a, b, c, d 都属于{1, 2, 3, 4}(2)a ≠b, b ≠c, c ≠d, d ≠a(3)a 是a, b, c, d 中的最小数那么,可以组成的不同的四位数abcd 的个数是________.12.46个。
abcd 中恰有2个不同数字时,能组成C 24=6个不同的数。
abcd 中恰有3个不同数字时,能组成1212121213C C C C C +=16个不同数。
abcd 中恰有4个不同数字时,能组成A 44=24个不同数,所以符合要求的数共有6+16+24=46个。
2012辽宁2012江苏9. 将27,37,47,48,55,71,75这 7个数排成一列,使任意连续4个数的和为3的倍数则这样的排法有____ 种。
解:将7个数分成3类:(1)3k 的数为 27,48,75,有3个(2)3k -1的数为47,71,有2个(3)3k +1的数为37,55,有2个要使排列的一列数中任意的四个数之和为3的倍数,则7个位置上第1位和第5位应排同一类数,第2和第6位排同一类数,第3和第7位排同一类数,且第4位必排第(1)类共有3种排法,三类数排到三类位置共有33A 种,每一类位置各有22A 种排法,故共有233233144A A =()种排法。
2012届高考数学一轮达标精品试卷(十一)排列组合二项式定理1

2012届高考数学一轮达标精品试卷(十一)第十一单元 排列组合、二项式定理(时量:120分钟 150分)一、选择题:本大题共18小题,每小题5分,共90分.在每小题给出的四个选项中,只有一项是符合题目要求的.1.5人排一个5天的值日表,每天排一人值日,每人可以排多天或不排,但相邻两天不能排同一人,值日表排法的总数为 A .120B .324C .720D .12802.一次考试中,要求考生从试卷上的9个题目中选6个进行答题,要求至少包含前5个题目中的3个,则考生答题的不同选法的种数是 A .40B .74C .84D .2003.以三棱柱的六个顶点中的四个顶点为顶点的三棱锥有 A .18个B .15个C .12个D .9个4.从一架钢琴挑出的十个音键中,分别选择3个,4个,5个,…,10个键同时按下,可发出和弦,若有一个音键不同,则发出不同的和弦,则这样的不同的和弦种数是 A .512B .968C .1013D .10245.如果()n x x x +的展开式中所有奇数项的系数和等于512,则展开式的中间项是 A .6810C xB .5710C xxC .468C xD .6811C xx6.用0,3,4,5,6排成无重复字的五位数,要求偶数字相邻,奇数字也相邻,则这样的五位数的个数是 A .36B .32C .24D .207.若n 是奇数,则112217777n n n n n n n C C C ---+++⋯⋯+被9除的余数是 A .0B .2C .7D .88.现有一个碱基A ,2个碱基C ,3个碱基G ,由这6个碱基组成的不同的碱基序列有 A .20个B .60个C .120个D .90个9.某班新年联欢会原定的6个节目已排成节目单,开演前又增加了3个新节目,如果将这3个节目插入原节目单中,那么不同的插法种数为 A .504B .210C .336D .12010.在342005(1)(1)(1)x x x ++++⋯⋯++的展开式中,x 3的系数等于A .42005CB .42006CC .32005CD .32006C11.现有男女学生共8人,从男生中选2人,从女生中选1人,分别参加数理化三科竞赛,共有90种不同方案,则男、女生人数可能是 A .2男6女B .3男5女C .5男3女D .6男2女12.若x ∈R ,n ∈N + ,定义nx M =x (x +1)(x +2)…(x +n -1),例如55M -=(-5)(-4)(-3)(-2)(-1)=-120,则函数199()x f x xM -=的奇偶性为A .是偶函数而不是奇函数B .是奇函数而不是偶函数C .既是奇函数又是偶函数D .既不是奇函数又不是偶函数13.由等式43243212341234(1)(1)(1)(1),x a x a x a x a x b x b x b x b ++++=++++++++定义映射12341234:(,,,)(,,,),f a a a a b b b b →则f (4,3,2,1)等于 A .(1,2,3,4)B .(0,3,4,0)C .(-1,0,2,-2)D .(0,-3,4,-1)14.已知集合A ={1,2,3},B ={4,5,6},从A 到B 的映射f (x ),B 中有且仅有2个元素有原象,则这样的映射个数为 A .8B .9C .24D .2715.有五名学生站成一排照毕业纪念照,其中甲不排在乙的左边,又不与乙相邻,而不同的站法有 A .24种B .36种C .60种D .66种16.等腰三角形的三边均为正数,它们周长不大于10,这样不同形状的三角形的种数为 A .8B .9C .10D .1117.甲、乙、丙三同学在课余时间负责一个计算机房的周一至周六的值班工作,每天1人值班,每人值班2天,如果甲同学不值周一的班,乙同学不值周六的班,则可以排出不同的值班表有 A .36种B .42种C .50种D .72种18.若1021022012100210139(2),()()x a a x a x a x a a a a a a -=+++⋯+++⋯+-++⋯+则 的值为 A .0B .2C .-1D .1答题卡题号 1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18答案二、填空题:本大题共6小题,每小题4分,共24分.把答案填在横线上.19.某电子器件的电路中,在A ,B 之间有C ,D ,E ,F 四个焊点(如图),如果焊点脱落,则可能导致电路不通.今发现A ,B 间电路不通,则焊点脱落的不同情况有 种. 20.设f (x )=x 5-5x 4+10x 3-10x 2+5x +1,则f (x )的反函数f -1(x )= .21.正整数a1a2…a n…a2n-2a2n-1称为凹数,如果a1>a2>…a n,且a2n-1>a2n-2>…>a n,其中a i (i=1,2,3,…)∈{0,1,2,…,9},请回答三位凹数a1a2a3(a1≠a3)共有个(用数字作答).22.如果a1(x-1)4+a2(x-1)3+a3(x-1)2+a4(x-1)+a5=x4,那么a2-a3+a4.23.一栋7层的楼房备有电梯,在一楼有甲、乙、丙三人进了电梯,则满足有且仅有一人要上7楼,且甲不在2楼下电梯的所有可能情况种数有.24.已知(x+1)6(ax-1)2的展开式中,x3的系数是56,则实数a的值为.三、解答题:本大题共3小题,共36分.解答应写出文字说明,证明过程或演算步骤.25.(本小题满分12分)将7个相同的小球任意放入四个不同的盒子中,每个盒子都不空,共有多少种不同的方法?26.(本小题满分12分)已知(41x+3x2)n展开式中的倒数第三项的系数为45,求:⑴含x3的项;⑵系数最大的项.27.(本小题满分12分)求证:123114710(31)(32)2.n n n n n n C C C n C n -++++⋯++=+⋅第十一单元 排列组合、二项式定理参考答案一、选择题(每小题5分,共90分): 题号 1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 答案DBCBBDCBABBADDBCBD提示1.D 分五步:5×4×4×4×4=1280.2.B 分三步:33425154545474.C C C C C C ++=3.C 46312.C -= 4.B 分8类:34510121012101010101010101010101010()2(11045)968.C C C C C C C C C C C +++⋯+=+++⋯+-++=-++=5.B 12512,10,n n -=∴=中间项为5555761010().T C x x C x x ==6.D 按首位数字的奇偶性分两类:2332223322()20A A A A A +-=7.C 原式=(7+1)n -1=(9-1)2-1=9k -2=9k ’+7(k 和k ’均为正整数).8.B 分三步:12365360C C C =9.A 939966504,504.A A A==或10.B 原式=11.B 设有男生x 人,则2138390,(1)(8)30x x C C A x x x -=--=即,检验知B 正确.12.A 2222()(9)(8)(9191)(1)(4)(81).f x x x x x x x x x =--⋯-+-=--⋯- 13.D 比较等式两边x 3的系数,得4=4+b 1,则b 1=0,故排除A ,C ;再比较等式两边的常数项,有1=1+b 1+b 2+b 3+b 4,∴b 1+b 2+b 3+b 4=0.14.D 223327.C =15.B 先排甲、乙外的3人,有33A 种排法,再插入甲、乙两人,有24A 种方法,又甲排乙的左边和甲排乙的右边各占12 ,故所求不同和站法有3234136().2A A =种16.C 共有(1,1,1),(1,2,2),(1,3,3),(1,4,4),(2,2,2),(2,2,3),(2,3,3),(2,4,4),(3,3,3)(3,3,4)10种.17.B 每人值班2天的排法或减去甲值周一或乙值周六的排法,再加上甲值周一且乙值周六的排法,共有2212264544242().C C A C A -+=种32003320062006442006(1)[1(1)](1)(1)(1).1(1)x x x x x x C x x+-+-+++=+-+即求中的系数为18.D 设f (x )=(2-x )10,则(a 0+a 2+…+a 10)2-(a 1+a 3+…+a 9)2=(a 0+a 1+…+a 10)(a 0-a 1+a 2-…-a 9+a 10)=f (1)f (-1)=(2+1)10(2-1)10=1。
2012-2019年新课标全国卷理——排列组合二项式定理

2012-2019年全国课标卷排列组合二项式定理题(2012年课标全国卷)2.将2名教师,4名学生分成2个小组,分别安排到甲、乙两地参加社会实践活动,每个小组由1名教师和2名学生组成,不同的安排方案共有( )A .12种B .10种C .9种D .8种(2013课标全国卷I )9、设m 为正整数,2()m x y +展开式的二项式系数的最大值为a ,21()m x y ++展开式的二项式系数的最大值为b ,若13a =7b ,则m = ( )A 、5B 、6错误!未找到引用源。
C 、7D 、8(2013年课标全国卷II )(5)已知(1+a x )(1+x )5的展开式中x 2的系数为5,则a =(A )- 4 (B )- 3(C )- 2 (D )- 1(2014年课标全国卷Ⅰ)13. 8()()x y x y -+的展开式中22x y 的系数为 .(用数字填写答案) (2014年课标全国卷Ⅱ)13.()10x a +的展开式中,7x 的系数为15,则a =________.(用数字填写答案) (2015年课标全国卷Ⅰ)(10)25()x x y ++的展开式中,52x y 的系数为(A )10 (B )20 (C )30 (D )60(2015年课标全国卷Ⅱ) (15)4()(1)a x x ++的展开式中x 的奇数次幂项的系数之和为32,则a =__________.(2016年课标全国卷Ⅰ)(14)5(2x +的展开式中,x 3的系数是 .(用数字填写答案) (2016年课标全国卷Ⅱ)(5)如图,小明从街道的E 处出发,先到F 处与小红会合,再一起到位于G 处的老年公寓参加志愿者活动,则小明到老年公寓可以选择的最短路径条数为( )(A )24 (B )18 (C )12 (D )9 (2016年课标全国卷Ⅲ)(12)定义“规范01数列”{}n a 如下:{}n a 共有2m 项,其中m 项为0,m 项为1,且对任意2k m ≤,12,,,k a a a 中0的个数不少于1的个数.若4m =,则不同的“规范01数列”共有( )(A )18个 (B )16个 (C )14个 (D )12个 (2017年课标全国卷Ⅰ)6.621(1)(1)x x++展开式中2x 的系数为 A .15 B .20 C .30 D .35(2017年课标全国卷Ⅱ)6. 安排3名志愿者完成4项工作,每人至少完成1项,每项工作由1人完成,则不同的安排方式共有( )A .12种B .18种C .24种D .36种(2017年课标全国卷Ⅲ)4.5()(2)x y x y +-的展开式中33x y 的系数为()A .-80B .-40C .40D .80(2018年课标全国卷Ⅰ)15.从2位女生,4位男生中选3人参加科技比赛,且至少有1位女生入选,则不同的选法共有_____________种.(用数字填写答案)(2018年课标全国卷Ⅱ)(2018年课标全国卷Ⅲ)5.522x x ⎛⎫+ ⎪⎝⎭的展开式中4x 的系数为 A .10 B .20 C .40 D .80 (2019年课标全国卷Ⅰ)(2019年课标全国卷Ⅱ)4.2019年1月3日嫦娥四号探测器成功实现人类历史上首次月球背面软着陆,我国航天事业取得又一重大成就,实现月球背面软着陆需要解决的一个关键技术问题是地面与探测器的通讯联系.为解决这个问题,发射了嫦娥四号中继星“鹊桥”,鹊桥沿着围绕地月拉格朗日2L 点的轨道运行.2L 点是平衡点,位于地月连线的延长线上.设地球质量为M 1,月球质量为M 2,地月距离为R ,2L 点到月球的距离为r ,根据牛顿运动定律和万有引力定律,r 满足方程: 121223()()M M M R r R r r R +=++. 设r Rα=,由于α的值很小,因此在近似计算中34532333(1)ααααα++≈+,则r 的近似值为ABCD(2019年课标全国卷Ⅲ)4.(1+2x 2 )(1+x )4的展开式中x 3的系数为A .12B .16C .20D .24。
太原理工大学研究生组合数学2012年期末考试
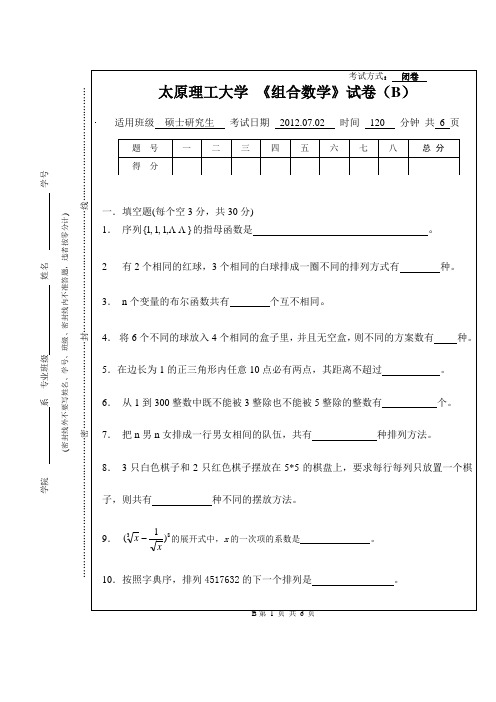
B 第 1 页 共 6 页 考试方式: 闭卷 太原理工大学 《组合数学》试卷(B ) 适用班级 硕士研究生 考试日期 2012.07.02 时间 120 分钟 共 6 页 一.填空题(每个空3分,共30分) 1. 序列},1,1,1{ 的指母函数是 。
2 有2个相同的红球,3个相同的白球排成一圈不同的排列方式有 种。
3. n 个变量的布尔函数共有 个互不相同。
4. 将6个不同的球放入4个相同的盒子里,并且无空盒,则不同的方案数有 种。
5.在边长为1的正三角形内任意10点必有两点,其距离不超过 。
6. 从1到300整数中既不能被3整除也不能被5整除的整数有 个。
7. 把n 男n 女排成一行男女相间的队伍,共有 种排列方法。
8. 3只白色棋子和2只红色棋子摆放在5*5的棋盘上,要求每行每列只放置一个棋子,则共有 种不同的摆放方法。
9. 83)1(x x 的展开式中,x 的一次项的系数是 。
10.按照字典序,排列4517632的下一个排列是 。
B 第 2 页 共 6 页二.(10分) 求解非齐次递推关系⎩⎨⎧==≥=+---1,02,3961021a a n a a a n n n nB 第 3 页 共 6 页三.(10分)用母函数求下式之和:n n n n n C n C C C 113121210+++++ .并给出组合意义。
四.(10分)正四面体每个面均为正三角形,现用红、蓝、黄,绿四种颜色为四个面着色,在空间转动能重合为同一着色方案。
问不同着色方案数为多少?五.(10分)求1,3,5,7,9这五个数可以组成多少个不同的n位数,其中要求3和7出现次数为偶数。
B第4 页共6 页B 第 5 页 共 6 页六.(10分)求正整数n=18900的因子个数。
并证明一整数是另一整数的平方的必要条件是它的因子数目为奇数。
七.(10分)求满足条件204321=+++x x x x ,511≤≤x ,702≤≤x ,623≤≤x ,844≤≤x 的整数解向量的个数。
2012组合教育密押三套卷全国卷(一)

密县(区) 姓名 准考证号考场号 科类密 封 线 内 不 得 答 题秘密★启用前2012年普通高等学校招生全国统一考试(全国卷)密押卷一数 学本试卷分第Ⅰ卷(选择题)和第Ⅱ卷(非选择题)两部分.满分150分,考试时间120分钟.第I 卷(选择题 共60分)一、选择题:本大题共12小题,每小题5分,共60分. 在每小题给出的四个选项中,只有一项是符合题目要求的. 1. (理)若i (为虚数单位),则的虚部是( ).()1i z z 1i i =+i A. B. C.D. 1−− (文)复数2i12i+=−i −2i 2i ( ). A. B. C. D. i − 2. 已知向量1,22⎛⎞=⎜⎟⎜⎟⎝⎠a ,1,22⎛⎞=−⎜⎟⎜⎟⎝⎠b ,k =+c a b =−d a b ∥c d 1c d 1k ,,如果,那么( ). A. 且与同向 B. k ==−c d 1c d 1k 且与同向 C. 且与反向 D. k ==−c d 30y 且与反向3. 已知直线:tan l x tan αβ−−=1的斜率为,在轴上的截距为,则2y ()tan αβ+=( ). A. 73−B. 73C. 571()3 D. 4. 执行如图所示的程序框图,如果依次输入函数xf x =,()sin f x x =,3()f x x =,1()f x x x+()f =,那么输出的函数x 3x sin 为( ).A. B. x C. 3x D. 1x x+012201218242736300 5. (理)“2012”含有数字,,,且有两个数字,则含有数字,,,且有两个相同数字的四位数的个数为( ). A. B. C. D. (文)如图是一个容量为的样本频率分布直方图,[则样本数据落在范围)13,1736707274的频数为( ). A. B. C. D.6. 已知变量x ,满足约束条件0,若目标函数x 仅在点处y 2303310x y x y y −+⎧⎪−+⎨⎪−⎩.--z y a =−()3,0−a 取得最大值,则实数的取值范围为( ). A. ()3,5 B. 1,2⎛⎞+∞⎜⎝⎠()1,2−⎟ C. D. 1,13⎛⎞⎜⎟⎝⎠1,0sgn()0,01,0x x x x >⎧⎪ 7. 已知符号函数==⎨⎪−<⎩()2()sgn ln ln ,则函数f x x x =−4321的零点个数为( ).A. B. C. D.8. 设椭圆224x y 1+=的左焦点为,F P ,则PF =( ). A.12 B. 32 C. 52 D. 729. 若函数>在区间(x )0()sin f x ωω=π0,3⎡⎤⎢⎣⎦⎥上单调递增,在区间ππ,32⎡⎤⎢⎥⎣⎦上单调递减,则ω=32( ).A. B. C. 32 D. 23c 0) 10. 设函数,若()0a ≠12()f x ax =+()(f x dx f x =∫,1,则00x --0x 的值为( ).A.12B. 4C. 2D. 3O 11. 球的球面上有四点,S A ,B ,,其中,C O A ,B ,四点共面,△是边长为 C ABC SAB S ABC 2的正三角形,平面平面,则棱锥⊥ABC −的体积的最大值为( ).A.B.13C. 2D. 3()2,11ax = 12. 点,,如果直线与线段()1,A 0B by +AB有一个公共点,那么a 222a b ++的( ). A. 最小值为45B. C. 最大值为45 D. 5第II 卷(非选择题 共90分)二、填空题:本大题共4小题,每小题分,共20分.13. 在△中,角ABC A ,B ,所对的边长分别为,,,若,C 120C ∠=a b c D c=,则 a b (填“”,“”或“”). ><=14. 下图是一个空间几何体的三视图,则该几何体的表面积是 .15. 已知以y =为渐近线的双曲线()2222:10,x y D a a b0b −=>>1F 2F P D 的左、右焦点分别为,,若为双曲线右支上任意一点,则112PF PF PF PF −+2的取值范围是 .16. 在数列111231,,2,,1,3,,,,4,23432⋅⋅⋅15中,(1)第项是 ;(2)1730在数列中第一次出现是第 项.三.解答题:本大题共6小题,共70分.解答应写出文字说明、证明过程或演算步骤. 17.(本小题满分12分)在△中,,,分别是角ABC ab c A ,B ,C 的对边,且满足.()2cos cos a c B b C −=(1)求角B 的大小; (2)若b =4,a c +=ABC P ABCD ,求△的面积.18.(理)(本小题满分12分)如图所示,已知四棱锥−的底面为菱形,且,ABCD 60ABC ∠=D PD AB 2PB ==PC =PA ,=,与相交于点. AC BD O PO (1)求证:⊥底面;ABCD PB PCD (2)求直线与平面所成角的正弦值; (3)若M 是上的一点,且PB CM PB ⊥,求PM的值. MB(文)(本小题满分12分) BCD 如图,在四棱锥P A −中,底面为直角梯形,ABCD AB CD ∥60DAB ∠=D 2AB CD ,,AD ==,且三角形为等腰三角形,. PAD PA PD =(1)求证:AD PB ⊥;(2)线段AP 上是否存在点M ,使得MD ∥PBC 平面?并说明理由.密封线密 封 线 内 不 得 答 题19.(本小题满分12分) (理)甲、乙、丙三人参加了一家公司的招聘面试,面试合格者可正式签约,甲表示只要面试合格就签约.乙、丙则约定:两人面试都合格就一同签约,否则两人都不签约.设甲面试合格的概率为1,乙、丙面试合格的概率都是21,且面试是否合格互不影响.3(1)求至少有1人面试合格的概率; (2)求签约人数ξ的分布列和数学期望.(文)(本小题满分12分)某学校为准备参加市运动会,对本校甲、乙两个田径队中30名跳高运动员进行了测试,并用茎叶图表示出本次测试30人的跳高成绩(单位:cm ).跳高成绩在以上(包括)定义为“合格”,成绩在以下(不包括)定义为“不合格”. 175cm 175c 175cmcm 178178c 186cm m C m 175(1)求甲队队员跳高成绩的中位数;(2)如果用分层抽样的方式从甲、乙两队所有的运动员中共抽取人,则人中“合格”与“不合格”的人数各为多少?m 55(3)从甲队以上(包括)选取两人,至少有一人在以上(包括)的概率为多少. cm 186c20.(本小题满分12分)(理)椭圆:()22221x y a b a b 0+=>>的离心率为2,长轴端点与短轴端点间的C .(1)求椭圆的方程;(2)设过点()0,D l C 4的直线与椭圆交于E ,两点,为坐标原点,若△直角三角形,求直线的斜率.F O OEFl (文)(本小题满分12分)已知椭圆M 的对称轴为坐标轴,且抛物线2x =−的焦点是椭圆M 的一个焦点,又点(A 在椭圆M 上. (1)求椭圆M 的方程;(2)已知直线的方向向量为l (,若直线l 与椭圆M 交于B 、两A 面C 点,求△积的最大值.BC21. (本小题满分12分)已知函数()()ln 1f x x a x =−−a ,∈R (). (1)讨论函数f x 1x .的单调性; (2)当时,ln ()1xf x x +-a 恒成立,求的取值范围.请考生在第(22)、(23)、(24)三题中任选一题做答,如果多答,则按题号小的记分,每道题满分10分).22.(本小题满分10分)选修4—1:几何证明选讲如图,已知圆上的弧pp AC C BD =,过点的圆的切线与BA 的延长线交于E 点,证明: (1);(2)ACE BCD ∠∠=2BC BE C =⋅D .23. (本小题满分10分)选修4—4:坐标系与参数方程os 已知曲线:1C 4c 3sin x t y t =−+=+t ⎧⎨⎩(为参数),:2C 8cos 3sin x y θθ==⎧⎨⎩(θ为参数).(1)化、的方程为普通方程,并说明它们分别表示什么曲线;1C C C P 2(2)若上的点对应的参数为1π2t =Q PQ ,为上的点,求的中点2C M 到直线:3C 322x t y t =+=−+t ⎧⎨⎩(为参数)距离的最小值.24.(本小题满分10分)选修4—5:不等式选讲设函数()3f x x a x =−+a a ,其中.0>(1)当1=时,求不等式()32f x x +.的解集; (2)若不等式的解集为()0-f x {}1x x −- ,求的值. a。
2012年高考数学备考之百所名校组合卷系列 专题01 集合与常用逻辑用语

2012年高考数学备考之百所名校组合卷系列一、选择题:1.(某某省某某市2012届高三教学质量测评文2)已知全集R,U = 集合{}1,2,3,4,5A =,[)2,B =+∞,则图中阴影部分所表示的集合为A . {0,1,2}B . {0,1}C . {1,2}D . {1} 【答案】D【解析】阴影部分的元素x A ∈且x B ∉,即UA B ⋂,选项D 符合要求.2.(某某省某某市鄞州区2012年3月高考适应性考试文科1)设集合{}{}032,4,2,0,22>--=-=x x x B A ,则=B C A U ( ){}0.A {}2.B {}2,0.C {}4,2,0.D【答案】C【解析】本题主要考查集合的概念与运算,一元二次不等式的解法。
{}31B x x x =><-或,{}13U C B x x =-≤≤,则=B C A U {}0,23. (某某省某某市鄞州区2012年3月高考适应性考试文科4)"6"πα=是"212cos "=α的( ).A 充分不必要条件 .B 必要不充分条件.C 充分必要条件.D 既不充分也不必要条件【答案】A【解析】本题主要考查简易逻辑中充分必要条件的判断。
当=6πα,则1cos 2=cos32πα=成立,但是1cos 22α=得到=,6k k Z παπ+∈不一定可以推出=6πα因此"6"πα=是"212cos "=α的充分不必要条件。
4.(某某省某某、天门等八市2012年3月高三联考理科1)设集合2{|03},{|320,}A x x B x x x x Z ==-+∈≤≤≤,则AB 等于A .(1,3)-B .[]1,2C .{}0,1,2D .{}1,2【答案】D【解析】{1,2}B =,{}1,2.AB =5.(某某省某某、天门等八市2012年3月高三联考理科4)下列命题中真命题的个数是①“2,0x R x x ∀∈->”的否定是“2,0x R x x ∃∈-<”; ②若|21|1x ->,则101x <<或10x<; ③*4,21x N x ∀∈+是奇数.A .0B .1C .2D .3【答案】C【解析】①错误,应为2"0"x x -≤;②正确,解|21|1x ->得1x >或0x <与“101x<<或10x<”等价;③正确. 6.(某某实验中学2012届高三第一次诊断性考试理1)设丨,则!等于()(A) .(B).(C).(D).7. (某某实验中学2012届高三第一次诊断性考试理5)下列有关命题的说法正确的是() (A) .命题"若,则X=1”的否命题为:“若,则;x 1”(B) "x=-l"是“”的必要不充分条件(C) .命题“^,使得:”的否定是:“,均有”(D) .命题“若x=y,则”的逆否命题为真命题【答案】D【解析】主要考查命题的否定形式,以及必要条件、充分条件与充要条件的判断,对于命题的否命题和否定形式要注意区分解:对于A :命题“若x 2=1,则x=1”的否命题为:“若x 2=1,则x≠1”.因为否命题应为“若x 2≠1,则x≠1”,故错误.对于B :“x=-1”是“x 2-5x-6=0”的必要不充分条件.因为x=-1⇒x 2-5x-6=0,应为充分条件,故错误.对于C :命题“∃x ∈R ,使得x 2+x+1<0”的否定是:“∀x ∈R ,均有x 2+x+1<0”. 因为命题的否定应为∀x ∈R ,均有x 2+x+1≥0.故错误. 由排除法得到D 正确.故答案选择D8. (某某省某某市2012年3月高中毕业班第二次调研测试理科1)设集合{2,1,0,1}A =--,{0,1,2,3,4}B =,则()A B =R A.∅ B.{0,1}C.{2,1}--D.{2,1,0,1}--【答案】C 【解析】AB R的意义是在集合A 中去掉属于集合B 的元素后余下的元素构成的集合,所以应当为{2,1}--.9.(市东城区2012届高三上学期期末考试文1)已知集合{}0A x x =≥,{}0,1,2B =,则(A )A B ⊆(B )B A ⊆(C )A B B ⋃=(D )A B ⋂=∅ 【答案】B【解析】由集合的子集定义,得B A ⊆。
2012高考数学试题分类汇编排列组合二项式定理

2012高考数学分类汇编-排列组合二项式定理1. (安徽7)2521(2)(1)x x+-的展开式的常数项是( ) ()A 3- ()B 2- ()C 2 ()D 3【解析】选D第一个因式取2x ,第二个因式取21x得:1451(1)5C ⨯-= 第一个因式取2,第二个因式取5(1)-得:52(1)2⨯-=- 展开式的常数项是5(2)3+-=2. (安徽10)6位同学在毕业聚会活动中进行纪念品的交换,任意两位同学之间最多交换一次,进行交换的两位同学互赠一份纪念品,已知6位同学之间共进行了13次交换,则收到4份纪念品的同学人数为( )()A 1或3 ()B 1或4 ()C 2或3 ()D 2或4【解析】选D261315132C -=-=①设仅有甲与乙,丙没交换纪念品,则收到4份纪念品的同学人数为2人②设仅有甲与乙,丙与丁没交换纪念品,则收到4份纪念品的同学人数为4人3.北京6.从0,2中选一个数字.从1.3.5中选两个数字,组成无重复数字的三位数.其中奇数的个数为( )A. 24B. 18C. 12D. 6【解析】由于题目要求的是奇数,那么对于此三位数可以分成两种情况:奇偶奇;偶奇奇。
如果是第一种奇偶奇的情况,可以从个位开始分析(3种选择),之后十位(2种选择),最后百位(2种选择),共12种;如果是第二种情况偶奇奇,分析同理:个位(3种情况),十位(2种情况),百位(不能是0,一种情况),共6种,因此总共12+6=18种情况。
【答案】B3.福建11.4)(x a +的展开式中3x 的系数等于8,则实数=a _________。
【2】考点:二项式定理。
难度:易。
分析:本题考查的知识点为二项式定理的展开式,直接应用即可。
解答:4)(x a +中含3x 的一项为r r r r x aC T -+=441,令3=r ,则83434=-a C ,即2=a 。
4.广东10. 261()x x +的展开式中3x 的系数为______。
2012全国数学高考题-排列组合

(5)一排9个座位坐了3个三口之家,若每家人坐在一起,则不同的坐法种数为(A)3×3! (B) 3×(3!)3 (C)(3!)4 (D) 9!【答案】C【解析】此排列可分两步进行,先把三个家庭分别排列,每个家庭有3!种排法,三个家庭共有33!3!3!(3!)⨯⨯=种排法;再把三个家庭进行全排列有3!种排法。
因此不同的坐法种数为4(3!),答案为C【点评】本题主要考查分步计数原理,以及分析问题、解决问题的能力,属于中档题。
(2012辽宁)11.将字母,,,,,a a b b c c 排成三行两列,要求每行的字母互不相同,每列的字母也互不相同,则不同的排列方法共有A .12种B .18种C .24种D .36种答案A【命题意图】本试题考查了排列组合的用用。
【解析】利用分步计数原理,先填写最左上角的数,有3种,再填写右上角的数为2种,在填写第二行第一列的数有2种,一共有32212⨯⨯=。
(2012全国)(11)现有16张不同的卡片,其中红色、黄色、蓝色、绿色卡片各4张,从中任取3张,要求这些卡片不能是同一种颜色,且红色卡片至多1张,不同取法的种数为(A )232 (B)252 (C)472 (D)484 解析:472885607216614151641122434316=-=--⨯⨯=--C C C C ,答案应选C 。
另解:472122642202111241261011123212143431204=-+=⨯⨯+-⨯⨯=+-C C C C C . (2012山东)8. 两人进行乒乓球比赛,先赢3局者获胜,决出胜负为止,则所有可能出现的情形(各人输赢局次的不同视为不同情形)共有( )(A ) 10种 (B )15种 (C ) 20种 (D ) 30种(2012陕西)11.三位同学参加跳高、跳远、铅球项目的比赛,若每人都选择其中两个项目,则有且仅有两人选择的项目完全相同的概率是 (结果用最简分数表示)。
2012年高考试题数学(文科)分项版之专题11 排列组合二项式定理

2012年高考试题数学(文科)分项版之专题11 排列组合二项式定理一、选择题1、(2012年高考四川卷文科11)方程22ay b x c=+中的,,{2,0,1,2,3}a b c∈-,且,,a b c互不相同,在所有这些方程所表示的曲线中,不同的抛物线共有()A、28条B、32条C、36条D、48条2、(2012年高考四川卷文科2)7(1)x+的展开式中2x的系数是()A、21B、28C、35D、423、(2012年高考全国卷文科7)6位选手依次演讲,其中选手甲不再第一个也不再最后一个演讲,则不同的演讲次序共有(A)240种(B)360种(C)480种(D)720种4、(2012年高考重庆卷文科4)5(13)x-的展开式中3x的系数为(A)-270 (B)-90 (C)90 (D)270二、填空题5、(2012年高考上海卷文科8)在61xx⎛⎫-⎪⎝⎭的二项式展开式中,常数项等于 .实用文档实用文档6、(2012年高考全国卷文科13)8)21(xx +的展开式中2x 的系数为____________.三、解答题7、(2012年高考江苏卷23)设集合{12}n P n =,,,…,n *∈N .记()f n 为同时满足下列条件的集合A 的个数: ①n A P ⊆;②若x A ∈,则2x A ∉;③若n P x A ∈,则2n P x A ∉.(1)求(4)f ;(2)求()f n 的解析式(用n 表示).以下是答案一、选择题1、 B【解析】方程22ay b x c =+变形得222b c y b a x -=,若表示抛物线,则0,0≠≠b a 所以,分b=-2,1,2,3四种情况: (1)若b=-2,⎪⎩⎪⎨⎧======2,1,033,1,0,23,2,0c ,1或或,或或或或c a c a a ; (2)若b=2, ⎪⎩⎪⎨⎧-==-===-=1,0,233,0,2c ,13,1,0,2或或,或或或或c a a c a实用文档2、3、C【解析】先排甲,有4种方法,剩余5人全排列有12055=A 种,所以不同的演讲次序有4801204=⨯种,选C.4、二、填空题5、实用文档 6、 7 【解析】二项展开式的通项为k k kk k k k x C x x C T )21()21(288881⨯==--+,令228=-k ,解得3=k ,所以2323847)21(x x C T =⨯=,所以2x 的系数为7.三、解答题7、【解析】(1)当=4n 时,符合条件的集合A 为:{}{}{}{}21,42,31,3,4,,,, ∴ (4)f =4.( 2 )任取偶数n x P ∈,将x 除以2 ,若商仍为偶数.再除以2 ,· 经过k 次以后.商必为奇数.此时记商为m 。
2012年高考数学备考之百所名校组合卷系列_专题03_数列

一、选择题:1. (广东省汕头市2012届高三教学质量测评文6)已知正项组成的等差数列{}n a 的前20项的和为100,那么615a a 的最大值为A .25B .50C .100D . 不存在【答案】A【解析】()()1202012020101002a a S a a +==+=,故12010a a +=,615120a a a a =2120252a a +⎛⎫≤= ⎪⎝⎭. 2.(湖北省荆门、天门等八市2012年3月高三联考理科3)如果数列1a ,21a a ,32a a ,…,1n n a a -,…是首项为1,公比为5a 等于A .32B .64C .32-D .64-【答案】A【解析】(10512343524511123432.a a a a a a a qa a a a +++=⨯⨯⨯⨯===3. (山东实验中学2012届高三第一次诊断性考试理4)已知{a n }为等差数列,其公差为-2,且a 7是a 3与a 9的等比中项,S n 为{an}的前n 项和,n ∈N*,则S 10的值为( )[21世纪教育网21世纪教育网 (A). -110 (B). -90 (C). 90 (D). 110【答案】D【解析】解:a 7是a 3与a 9的等比中项,公差为-2,所以a 72=a 3•a 9,所以a 72=(a 7+8)(a 7-4),所以a 7=8,所以a 1=20,所以S 10= 10×20+10×9/2×(-2)=110。
故选D4. (湖南省衡阳八中2012届高三第三次月考理科3)公差不为零的等差数列第2,3,6项构成等比数列,则A .1B .2C .3D .4【答案】C【解析】设公差为d ,由题意知:2326a a a =,即2111(2)()(5)a d a d a d +=++,解得12d a =-,所以公比为32a a =112a d a d+=+3,选C.5. (湖南省衡阳八中2012届高三第三次月考理科6)已知各项均为正数的等比数列{}n a ,1235a a a =,456a a a =789a a a =A .10B .C . 8 D【答案】A【解析】因为123,a a a 456,a a a 789a a a 成等比数列,所以789a a a =10,选A.6. (山东省青岛市2012届高三上学期期末检测理科7)等差数列{}n a 中,已知16a =-,0n a =,公差d ∈N *,则n ()3n ≥的最大值为A .7B .6C .5D .8答案:A解析:1(1)0n a a n d =+-=,∴61d n =-,又d ∈N *,∴n ()3n ≥的最大值为7.二、填空题:7.(北京市东城区2012届高三上学期期末考试文12)在等差数列{}n a 中,若57684,2a a a a +=+=-,则数列{}n a 的公差等于 ; 其前n 项和n S 的最大值为 . 【答案】57【解析】57686714,2,2,1,3,17a a a a a a d a +=+=-∴==-∴=-= ,所以 前6项的和最大1666()572a a S +==8. (福建省泉州市2012年3月普通高中毕业班质量检查理科11)已知等差数列}{n a 中, 51a =,322a a =+,则11S = .【解析】62,3,d a ==()111116111133.2a a S a +===9. (湖南省衡阳八中2012届高三第三次月考理科11)正项数列{}n a 满足12a =,21(2)8(2)n n a S n --=≥,则{}n a 的通项公式为n a = . 【答案】42n -【解析】因为21(2)8(2)n n a S n --=≥,所以21(2)8n n a S +-=,两式相减得:2211844n n n n n a a a a a ++=-+-,整理得: 1114()()()n n n n n n a a a a a a ++++=-+,因为{}n a 是正项数列,所以14n n a a +-=,所以{}n a 是以4为公差,2为首项的等差数列, 所以24(1)n a n =+-=42n -.10. (湖南省衡阳八中2012届高三第三次月考理科15) 当n 为正整数时,定义函数()N n 表示n 的最大奇因数.如(3)3,(10)5N N ==,….记()(1)(2)(3)(2)n S n N N N N =++++ . 则(1)(4)S = .(2)()S n = .【答案】86;423n+【解析】由题设知,(2)(),(21)21N n N n N n n =-=-.(1)(4)[(1)(3)(5)(15)][(2)(4)(6)(16)],S N N N N N N N N =+++++++++[13515][(2)(4)(6)(16)]N N N N =+++++++++3323214(3)44(2)444(1)S S S =+=++=+++86=.(2)()[135(21)][(2)(4)(6)(2)],nnS n N N N N =++++-+++++11()4(1)(1),(1)1n S n S n n S N -∴=+-≥==又,12142()444413nn n S n --+∴=+++++=.11. (浙江省宁波市鄞州区2012年3月高考适应性考试文科16)对于正项数列{}n a ,定义nn na a a a nH ++++=32132,若,22+=n H n 则数列n a 的通项公式为 .【答案】nn 212+【解析】本题主要考查数列通项公式的求法的问题。
2012年高考数学备考之百所名校组合卷系列 专题11 选修系列

2012年高考数学备考之百所名校组合卷系列一、填空题:1.(某某省某某市2012届高三教学质量测评文14)(坐标系与参数方程选做题)过点(2,)3π且平行于极轴的直线的极坐标方程为.【答案】sin ρθ=【解析】先将极坐标化成直角坐标表示,(2,)3π化为,过且平行于x 轴的直线为y =sin ρθ=法二:在极坐标系中直接构造直角三角形由其边角关系得方程sin ρθ=2.(某某省某某市2012届高三教学质量测评文15)(几何证明选讲选做题)已知PA 是O 的切线,切点为A ,直线PO 交O 于B 、C 两点,2AC =,120PAB ∠=︒,则O 的面积为.【答案】4π【解析】由弦切角定理,PAC ABC ∠=∠,由120PAB ∠=︒,90CAB ∠=︒得30PAC ABC ∠=∠=︒,在Rt ABC ∆中,22224R BC AC ===⨯=,4R =,2S R π==4π.3.(某某省某某中学、海门中学、天一中学2012届高三联考11)已知变量,a R θ∈,则22(2cos )(2sin )a a θθ-+-的最小值为.【解析】22(2cos )(2sin )a a θθ-+-的几何意义为(),a a到()2cos 2sin θθ距离的平方。
(),a a 表示的轨迹为y x =,()2cos 2sin θθ表示的轨迹为(224x y +-=又(到y x =是距离为5,所以(),a a到()2cos 2sin θθ+距离最小值为3。
所以22(2cos )(2sin )a a θθ-+-的最小值为9.三、解答题4.(某某省某某市2012年3月高中毕业班第二次调研测试理科22)(本小题满分10分)选修4-1:几何证明选讲.如图,在△ABC 中,CD 是ACB ∠的平分线,△ACD 的外接圆交BC 于点E ,2AB AC =.⑴求证:2BE AD =; ⑵当1AC =,2EC =时,求AD 的长.【解析】⑴连结DE ,因为ACED 是圆的内接四边形,所以BDE BCA ∠=∠,又DBE CBA ∠=∠,所以△BDE ∽△BCA ,即有BE DEBA CA=,而2AB AC =, 所以2BE DE =.又CD 是ACB ∠的平分线,所以AD DE =,从而2BE AD =.⑵由条件得22AB AC ==,设AD t =,根据割线定理得 BD BA BE BC ⋅=⋅,即()2(2)AB AD BA AD AD CE -⋅=⋅+PABOC(第15题图)所以(2)22(22)t t t -⨯=+,即22320t t +-=,解得12t =,即12AD =. 5. (某某省某某市2012年3月高中毕业班第二次调研测试理科23)(本小题满分10分)选修4-4:坐标系与参数方程选讲.在直角坐标系xOy 中,曲线1C 的参数方程为cos 1sin x y αα=⎧⎨=+⎩(α为参数).以O 为极点,x 轴正半轴为极轴,建立极坐标系.曲线2C 的极坐标方程为(cos sin )10ρθθ-+=.⑴求曲线1C 的普通方程和2C 的直角坐标方程; ⑵求曲线1C 上的点到曲线2C 的最远距离.【解析】⑴将cos 1sin x y αα=⎧⎨=+⎩(α为参数)化为普通方程得()1122=-+y x ,将()cos sin 10ρθθ-+=化为直角坐标方程得01=+-y x .⑵ 由⑴知曲线1C 表示圆心为(0,1),半径为1的圆,曲线2C 表示直线01=+-y x ,并且过圆心(0,1),所以曲线1C 上的点到曲线2C 上点的最远距离等于圆的半径1. 6. (某某省某某市2012年3月高中毕业班第二次调研测试理科24)(本小题满分10分)选修4-5:不等式选讲.设函数|32||12|)(-+-=x x x f ,∈x R .⑴解不等式()5f x ≤;⑵若mx f x g +=)(1)(的定义域为R ,某某数m 的取值X 围.【解析】⑴原不等式等价于12445x x ⎧<⎪⎨⎪-⎩≤或132225x ⎧⎪⎨⎪⎩≤≤≤或32445x x ⎧>⎪⎨⎪-⎩≤,因此不等式的解集为]49,41[-∈x .⑵由于mx f x g +=)(1)(的定义域为R ,则0)(=+m x f 在R 上无解.又()|21||23||2123|2f x x x x x =-+---+=≥,)(x f 的最小值为2,所以2m -<,即2m >-.7. (某某省某某中学、海门中学、天一中学2012届高三联考21)A 选修4-2:矩阵与变换(本小题满分10分)已知矩阵1121⎡⎤=⎢⎥⎣⎦A ,向量12β⎡⎤=⎢⎥⎣⎦.求向量α,使得2αβ=A . 【解析】:1121⎡⎤=⎢⎥⎣⎦A ,2111132212143⎡⎤⎡⎤⎡⎤∴==⎢⎥⎢⎥⎢⎥⎣⎦⎣⎦⎣⎦A ………………4分 设x y α⎡⎤=⎢⎥⎣⎦,则2αβ=⇔A 3243⎡⎤⎢⎥⎣⎦x y ⎡⎤⎢⎥⎣⎦=12⎡⎤⎢⎥⎣⎦⇔321432x y x y +⎡⎤⎡⎤=⎢⎥⎢⎥+⎣⎦⎣⎦…………8分 3211,4322x y x x y y +==-⎧⎧∴∴⎨⎨+==⎩⎩,12α-⎡⎤∴=⎢⎥⎣⎦. ………………10分 8. (某某省某某中学、海门中学、天一中学2012届高三联考21)B 选修4-4:坐标系与参数方程(本小题满分10分)在直角坐标系xoy 中,直线l 的参数方程为122322x t y t ⎧=⎪⎪⎨⎪=+⎪⎩(t 为参数),若以直角坐标系xoy 的O 点为极点,ox 为极轴,且长度单位相同,建立极坐标系,得曲线C 的极坐标方程为2cos()4πρθ=-.(1)求直线l 的倾斜角;(2)若直线l 与曲线C 交于,A B 两点,求AB .9.(某某省某某市2012年3月普通高中毕业班质量检查理科21)(本小题满分7分)选修4—2:矩阵与变换若二阶矩阵M 满足127103446M ⎛⎫⎛⎫= ⎪ ⎪⎝⎭⎝⎭. (Ⅰ)求二阶矩阵M ;(Ⅱ)把矩阵M 所对应的变换作用在曲线223861x xy y ++=上,求所得曲线的方程.【解析】(Ⅰ)记矩阵1234A ⎛⎫= ⎪⎝⎭,故2A =-,故1213122A --⎛⎫ ⎪= ⎪-⎝⎭. 由已知得121710710123146461122M A --⎛⎫⎛⎫⎛⎫⎛⎫ ⎪=== ⎪ ⎪ ⎪ ⎪-⎝⎭⎝⎭⎝⎭⎝⎭. (Ⅱ)设二阶矩阵M 所对应的变换为1211x x y y '⎛⎫⎛⎫⎛⎫= ⎪ ⎪⎪'⎝⎭⎝⎭⎝⎭,得2x x yy x y '=+⎧⎨'=+⎩, 解得2x x y y x y ''=-+⎧⎨''=-⎩,又223861x xy y ++=,故有223(2)8(2)()6()1x y x y x y x y ''''''''-++-+-+-=,化简得2221x y ''+=.故所得曲线的方程为2221x y +=.10.(某某省某某市2012年3月普通高中毕业班质量检查理科21)(本小题满分7分)选修4-4:坐标系与参数方程已知在直角坐标系xOy 中,曲线C 的参数方程为2cos 2sin x t y θθ=⎧⎨=⎩(t 为非零常数,θ为参数),在极坐标系(与直角坐标系xOy 取相同的长度单位,且以原点O 为极点,以x 轴正半轴为极轴)中,直线l 的方程为sin()4πρθ-=(Ⅰ)求曲线C 的普通方程并说明曲线的形状;(Ⅱ)是否存在实数t ,使得直线l 与曲线C 有两个不同的公共点A 、B ,且10OA OB ⋅=(其中O 为坐标原点)?若存在,请求出;否则,请说明理由.【解析】本题主要考查曲线的参数方程、直线的极坐标方程等基础知识,考查运算求解能力以及化归与转化思想、分类与整合思想.满分7分.解:(Ⅰ)∵0t ≠,∴可将曲线C 的方程化为普通方程:2224x y t+=.①当1t =±时,曲线C 为圆心在原点,半径为2的圆; ②当1t ≠±时,曲线C 为中心在原点的椭圆. (Ⅱ)直线l 的普通方程为:40x y -+=.联立直线与曲线的方程,消y 得222(4)4x x t++=,化简得2222(1)8120t x t x t +++=.若直线l 与曲线C 有两个不同的公共点,则422644(1)120t t t ∆=-+⋅>,解得23t >.又22121222812,,11t t x x x x t t +=-=++ 故12121212(4)(4)OA OB x x y y x x x x ⋅=+=+++121224()1610x x x x =+++=.解得23t =与23t >相矛盾. 故不存在满足题意的实数t .11.(某某省某某市2012年3月普通高中毕业班质量检查理科21)(本小题满分7分)选修4—5:不等式选讲已知函数()24f x x x =-+-的最小值为m ,实数,,,,,a b c n p q 满足222222a b c n p q m ++=++=.(Ⅰ)求m 的值;(Ⅱ)求证:4442222n p q a b c++≥.【解析】本题主要考查绝对值的几何意义、柯西不等式等基础知识,考查运算求解能力以及推理论证能力,考查函数与方程思想以及分类与整合思想.满分7分.解:(Ⅰ)法一: 26(4)()242(24)26(2)x x f x x x x x x -≥⎧⎪=-+-=<<⎨⎪-+≤⎩, 可得函数的最小值为2.故2m =.法二:()24(2)(4)2f x x x x x =-+-≥---=, 当且仅当24x ≤≤时,等号成立,故2m =.(Ⅱ) 222222222[()()()]()n p q a b c a b c++⋅++2222()n p q a b c a b c ≥⋅+⋅+⋅……5分即:444222()2n p q a b c ++⨯≥2222()4n p q ++=,故4442222n p q a b c++≥.12.(某某省某某市、某某市2012届高三第一次模拟21)13.B.(某某省某某市、某某市2012届高三第一次模拟)(选修4—2:矩阵与变换)已知矩阵1101,20201⎡⎤⎡⎤⎢⎥==⎢⎥⎢⎥⎣⎦⎣⎦A B,若矩阵AB对应的变换把直线l:20x y+-=变为直线'l,求直线'l的方程.【解析】C 的方程化为4cos 4sin ρθθ=+,两边同乘以ρ,得24cos 4sin ρρθρθ=+由222,cos ,sin x y x y ρρθρθ=+== ,得22440x y x y +--=……………5分 其圆心C 坐标为(2,2),半径22r =又直线l 的普通方程为20x y --=, ∴圆心C 到直线l 的距离22d ==∴弦长28226AB =-=10分 D. (某某省某某市、某某市2012届高三第一次模拟)(选修4—5:不等式选讲) 已知x y z 、、2223111111()x y zx y z++≤++. 【解析】证明:由柯西不等式得2222222111111(111)()()x y z x y z++++≥++……………5分 2221111113x y z x y z ++≥++,即2223111111()3x y z x y z++≤++……10分【点评】作为选作题部分,只要求学生掌握相应的基础知识即可,一般试题的难度相对基础,侧重基本知识,基本技能的考查,考生在选作的时候应该选择自己最优把握的试题进行规X 解答。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
15. (3’) There is a party with 4 couples. In how many ways can they choose their partner, where each pair of
The number of ways to place n non-attacking, indistinguishable rooks on an n-by-n board with forbidden positions equals n! – r1(n-1)! + r2(n-2)! - ... + (-1)k rk(n-k)!+…+(-1)rrn. For a specified grid i in chessboard C, let Ci be a chessboard induced from C by deleting the row and the column of grid i;let Ce be induced from C by deleting grid i from C. Then, rk(C)=rk-1(Ci)+rk(Ce),R(C)= xR(Ci) + R(C e). Assume that the sequence h0, h1, h2, …, hn,… has a difference table whose 0th diagonal equals, c0, c1, c2, …, cp≠0, 0, 0, … Then, hn = c0 C(n, 0) + c1C(n, 1) + c2C(n, 2) + … +cpC(n, p). and
3. (3’) Consider the permutation of {1,2,3,4,5,6,7,8}. The inversion sequence of 83476215 is
( ); The permutation with an inversion sequence 66142100 is ( ).
4. (3’) In which position does the combination 1289 occur in the lexicographic order of the 4-combinations of
{1, 2, 3, 4, 5, 6, 7, 8, 9}? ( ).
5. (3’) Among the combination of {x7, x6, …, x1, x0}, what is the combination that immediately follow {x7, x5,
preferential ranking matrix a ������ ������ ������ ������ 1,3 1,4 2,2 4,1 b 2,3 4,1 1,4 2,2 c 3,2 3,3 3,4 3,1 d 4,3 2,2 4,1 1,4
Page No. 2
Question Two (10’): Let A = {A1 , A2 , A3 , A4 , A5 , A6 },where A1 = {a, b, c}, A2 = {a, b, c, d, e}, A3 = {a, b}, A4 = {b, c}, A5 = {a}, A6 = {a, c, e}. Does A has SDRs ? Why? What is the largest number of sets of A which can be chosen such that they have an SDR?
x4, x3, x2, x1, x0} by using the base 2 arithmetic generating scheme? ( )
6. (3’) In how many ways can six women, eight men and a dog sit around a table such that no two women sit
Then the number of ways is ( ways is ( ). ). If the balls are distinguishable, then the number of
14. (3’) In how many ways can 5 red rooks and 3 blue rooks can be placed on 10×10 board so that no two
using the colors red, green, and black so that no two squares that are colored red are adjacent. Then, the recurrence relation for hn is ( ).
PG ( z1 , z2 ,, zn )
there are two whose distance apart is at most n .
1
The following formula and results may be useful for you in this examination.
mn = (
ห้องสมุดไป่ตู้
)
1
x2 x4 1 (e x e x ) 2! 4! 2 2 x x ex 1 1! 2! xn e ax a n n! n 0
n k=0 hk
=(
).
12. (3’) Let
1 2 3 4 5 6 f= 6 4 2 1 5 3 and g = 1 2 3 4 5 3 5 6 2 4 6 1
Note: Please give a detailed explanation for the following Five problems. Question One (6’): Prove that, among any 50 points in a square of size 7cm × 7cm, there are two points whose distance apart is at most 2cm.
Let c=(R, B, B, R, R, R) be a coloring of 1, 2, 3, 4, 5, 6 with the colors R and B. Then (g∘ f)∗ c = ( ) and (f∘ g)∗ c = ( )
13. (3’) Suppose 20 indistinguishable balls are allocated to 5 persons so that everyone has no less than 3 balls.
N (G, C ) 1 | C( f ) | . | G | f G
7. (3’) How many circular permutations are there of the multiset {3· a, 4· b, 2· c, 1· d}, where for each type of
partners is not couples?
16. (3’) Count the permutations i1 , i2 , i3 , i4 , i5 , i6 of {1,2,3,4,5,6} where i2 ≠ 1,4 ; i3 ≠ 2,3,5 , i4 ≠ 3,4 ,
i6 ≠ 5,6
17. (3’) Apply the deferred acceptance algorithm to obtain a stable complete marriage for the following
e3 + 6e4 = n.
Page No. 1
10. (3’) Let hn equal the number of different ways in which the squares of a 1-by-n chessboard can be
colored, using the colors red, blue, white, and green, so that the number of squares colored red is even, and the number of squares colored white is odd. Then hn = ( ).
1. (3’) A football team of 11 players is to be selected from a set of players, 5 of whom can play only in the
backfield, 8 of them can only play on the line, 2 of them can play either in the backfield, or on the line. Assuming a football team has 7 men on the line and 4 men in the backfield, the number of possible football
18. (3’) Complete the following semi-Latin square or order 5.
11. (3’) Let the sequence h0 , h1 , h2 , … hn … be defined by hn = 2n2 − n + 3, (n ≥ 0).
Then
letters, all letters of that type do not appear consecutively.
