复变函数试题A

合集下载

2011.9-2012.1复变函数试卷--A答

2011.9-2012.1复变函数试卷--A答得分评阅人一、判断分析题（要求写出充分的理由.每小题4分，共8分）1.函数22()f z xy ix y =+在z 平面上处处解析。

解答：该命题错误。

记2(,)u x y xy =，2(,)v x y x y =，显然它们在平面上具有连续的偏导数，且2u y x ?=?，2u xy y ?=?，2vxy x ?=? ，2v x y=? 要使柯西—黎曼条件条件满足，只须 22u vy x x y===??，22u v xy xy y x==-=-??，即0x =，0y =故此函数仅在点0z =可导，而在复平面上处处不解析．2.0=z 是函数)/1sin(1)(z z f =的孤立奇点。

解答：该命题错误。

因为)/1sin(1)(z z f =的奇点有,...)2,1,0(1,0±±===k k z z π，所以在0=z 的任意去心邻域内，总包括奇点πk z 1=，当∞→k 时，0=z 。

从而0=z 不是)/1sin(1)(z z f =的孤立奇点。

3.函数sin z 在z 平面上是有界的.解答：该命题错误。

…………………………1分sin z 在z 平面上无界。

这是因为sin 2iz ize e z i --=，令(0)z iy y =<，则|sin |||()2iz ize e z y i--=→∞→-∞…3分得分评阅人二、填空题（将正确的内容填在各题干预备的横线上，内容填错或未填者，该空无分.共8小题，每小题2分，共16分）1. 设i z 43+-=，则arg z =32arctan-π. 2. i +12=,...1,0),2ln sin 2ln (cos )22(ln ±=+-k i e k π.3．若C 是单位圆周，n 是自然数，则=-?C ndz z z )(10??∈≠∈=D z D z n Dz n i 000,01,0,1,2，π．或者??≠∈=Dz n Dz n i 00,1,0,1,2π 4．幂级数∑+∞=02n nz n 的收敛半径为 =R 1 .幂级数12nn n nz ∞=∑的收敛半径R = 2 .5．函数)(z f 在区域D 内解析是指 )(z f 在区域D 内每一点可导 . 6．在扩充复平面上亚纯函数在各奇点的残（留）数之和为_0__. 7．指数函数z e ω=的基本周期为i π2.8. 设 2sin iw e π=，则 =)Re(w 0 .9. ()f z ，()g z 分别以z a =为m 级极点与n 级极点，则z a =为()()f zg z 的m n -级极点()m n >，n m -级零点()m n <，可去奇点()m n =. 得分评阅人三、单项选择题（将正确的内容填在各题干预备的横线上，内容填错或未填者，该空无分.共8小题，每小题2分，共16分）1．区域12z <<的边界是1z =，2z =，它们的正方向( B ).(A)1z =，2z =都是“逆时针” (B)1z =“顺时针”，2z =“逆时针” (C)1z =，2z =都是“顺时针” (D)1z =“逆时针”，2z =“顺时针” 2．设)(z f 在单连通区域D 内解析, L 为D 内一条简单闭曲线, 则必有（ D ）.A ．2 Im[()]d 0.Lf z z =? B ．2 Re[()]d 0.Lf z z =?C ．2()d 0.Lf z z =?D ．2()d 0.Lf z z =?3．()f z 的孤立奇点a 为本性奇点的充要条件是( B ).A ．lim ()0z af z →= B ．lim ()z af z →不存在 C ．lim ()()z af z b →=≠∞ D ．lim ()z af z →=∞4．设32z i =--，则arg z =( C ). A ． 2ar 3ctgB ． 3ar 2ctgC ． 2ar 3ctg π-D ． 2ar 3ctg π+ 5．设()f z 在1z <内除三个五级极点外解析，并有四个四级零点，在1z =时解析且无零点，则1()()z f z dz f z ='=?( B ). A ．2i π- B ．2i π C ．1- D ．1 6．69)4sin 4(cos )sin (cos θθθθ?i i ei +-=，则?=( A ). A ．θ33- B ．θ15- C ．θ15 D ．θ33 7．设C 为不经过点与-1的正向简单闭曲线，则?+-c z z zdz2)1)(1(为( D ).A ．2iπ B ．i-π C ．0 D ． A 、B 、C 都有可能 8．设)(z f 在区域D 内解析，C 为D 内任一条正向简单闭曲线，它的内部全属于D ．如果)(z f 在C 上的值为9，那么对C 内任一点)(,00z f z ( C ).A ．等于10B ．等于0C ．等于9D ．919. 复级数11()n n n n n a a ib ∞∞===+∑∑收敛的充要条件是( C ).A ．0n a →B ．1n n a ∞=∑收敛 C ．实级数1n n a ∞=∑及1n n b ∞=∑皆收敛D ．实级数1n n a ∞=∑及1n n b ∞=∑至少有一个收敛得分评阅人四、计算题（共5小题，每小题9分，共45分）1．设)(z f 在1||z dz z f zz z )()]1(4[12?=+±.解：z dz z f z z z )()]1(4[1||22?=+±=dz zz f z zf z z f z ?=±±1||3)])()()(4[……………4分 =)0(8]2)0(4[2]2)0()0(4[2f i i f i f f i ''±=''±=''±ππππ………4分2. 试求函数zz z z f 6tan )(2π-=的所有有限孤立奇点，并判断它们的类型。

复变函数期末试题及答案

复变函数期末试题及答案一、选择题（每题5分，共20分）1. 若复数 $ z = a + bi $（其中 $ a, b $ 为实数），则$ \bar{z} $ 表示（）A. $ a - bi $B. $ -a + bi $C. $ -a - bi $D. $ a + bi $答案：A2. 对于复变函数 $ f(z) = u(x, y) + iv(x, y) $，以下说法正确的是（）A. $ u $ 和 $ v $ 都是调和函数B. $ u $ 和 $ v $ 都是解析函数C. $ u $ 和 $ v $ 都是连续函数D. $ u $ 和 $ v $ 都是可微函数答案：A3. 若 $ f(z) $ 在 $ z_0 $ 处可导，则下列说法中正确的是（）A. $ f(z) $ 在 $ z_0 $ 处解析B. $ f(z) $ 在 $ z_0 $ 处连续C. $ f(z) $ 在 $ z_0 $ 处可微D. $ f(z) $ 在 $ z_0 $ 处的导数为0答案：C4. 已知 $ f(z) $ 是解析函数，且 $ f(z) $ 在 $ z_0 $ 处有孤立奇点，则 $ f(z) $ 在 $ z_0 $ 处的留数是（）A. 0B. $ \infty $C. 1D. $ -1 $答案：A二、填空题（每题5分，共20分）1. 若 $ z = x + yi $，且 $ |z| = 2 $，则 $ x^2 + y^2 = \_\_\_\_\_ $。

答案：42. 设 $ f(z) = z^2 $，则 $ f(2 + 3i) = \_\_\_\_\_ $。

答案：-5 + 12i3. 若 $ f(z) $ 在 $ z_0 $ 处解析，则 $ f(z) $ 在 $ z_0 $ 处的导数 $ f'(z_0) $ 等于 \_\_\_\_\_。

完整版)复变函数测试题及答案

完整版)复变函数测试题及答案复变函数测验题第一章复数与复变函数一、选择题1.当 $z=\frac{1+i}{1-i}$ 时，$z+z+z$ 的值等于（）A) $i$ (B) $-i$ (C) $1$ (D) $-1$2.设复数 $z$ 满足 $\operatorname{arc}(z+2)=\frac{\pi}{3}$，$\operatorname{arc}(z-2)=\frac{5\pi}{6}$，那么 $z$ 等于（）A) $-1+3i$ (B) $-3+i$ (C) $-\frac{2}{3}+\frac{2\sqrt{3}}{3}i$ (D) $\frac{1}{3}+2\sqrt{3}i$3.复数 $z=\tan\theta-i\left(\frac{1}{2}\right)$，$0<\theta<\pi$，则 $[0<\theta<\frac{\pi}{2}$ 时，$z$ 的三角表示式是（）A) $\sec\theta[\cos(\pi+\theta)+i\sin(\pi+\theta)]$ (B)$\sec\theta[\cos\theta+i\sin\theta]$ (C) $-\sec\theta[\cos(\pi+\theta)+i\sin(\pi+\theta)]$ (D) $-\sec\theta[\cos\theta+i\sin\theta]$4.若 $z$ 为非零复数，则 $z^2-\bar{z}^2$ 与$2\operatorname{Re}(z)$ 的关系是（）A) $z^2-\bar{z}^2\geq 2\operatorname{Re}(z)$ (B) $z^2-\bar{z}^2=2\operatorname{Re}(z)$ (C) $z^2-\bar{z}^2\leq2\operatorname{Re}(z)$ (D) 不能比较大小5.设 $x,y$ 为实数，$z_1=x+1+\mathrm{i}y,z_2=x-1+\mathrm{i}y$ 且有 $z_1+z_2=12$，则动点 $(x,y)$ 的轨迹是（）A) 圆 (B) 椭圆 (C) 双曲线 (D) 抛物线6.一个向量顺时针旋转 $\frac{\pi}{3}$，向右平移 $3$ 个单位，再向下平移 $1$ 个单位后对应的复数为 $1-3\mathrm{i}$，则原向量对应的复数是（）A) $2$ (B) $1+3\mathrm{i}$ (C) $3-\mathrm{i}$ (D)$3+\mathrm{i}$7.使得 $z=\bar{z}$ 成立的复数 $z$ 是（）A) 不存在的 (B) 唯一的 (C) 纯虚数 (D) 实数8.设 $z$ 为复数，则方程 $z+\bar{z}=2+\mathrm{i}$ 的解是（）A) $-\frac{3}{3}+\mathrm{i}$ (B) $-\mathrm{i}$ (C)$\mathrm{i}$ (D) $-\mathrm{i}+4$9.满足不等式$|z+i|\leq 2$ 的所有点$z$ 构成的集合是（）A) 有界区域 (B) 无界区域 (C) 有界闭区域 (D) 无界闭区域10.方程 $z+2-3\mathrm{i}=2$ 所代表的曲线是（）A) 中心为 $2-3\mathrm{i}$，半径为 $2$ 的圆周 (B) 中心为 $-2+3\mathrm{i}$，半径为 $2$ 的圆周 (C) 中心为 $-2+3\mathrm{i}$，半径为 $2$ 的圆周 (D) 中心为 $2-3\mathrm{i}$，半径为 $2$ 的圆周11.下列方程所表示的曲线中，不是圆周的为（）A) $\frac{z-1}{z+2}=2$ (B) $z+3-\bar{z}-3=4$ (C) $|z-a|=1$ ($a0$)12.设 $f(z)=1-z$，$z_1=2+3\mathrm{i}$，$z_2=5-\mathrm{i}$，则 $f(z_1-z_2)$ 等于（）A) $-2-2\mathrm{i}$ (B) $-2+2\mathrm{i}$ (C)$2+2\mathrm{i}$ (D) $2-2\mathrm{i}$1.设 $f(z)=1$，$f'(z)=1+i$，则 $\lim_{z\to 0}\frac{f(z)-1}{z}=$ $f(z)$ 在区域 $D$ 内解析，且 $u+v$ 是实常数，则$f(z)$ 在 $D$ 内是常数。

复变函数复习资料

《复变函数》试卷一、单项选择题1．以下命题正确的是[ A ]A ．1z iz i =B ．零的辐角为零C ．3i i <D ．对任意复数z 有sin 1z ≤2．若1(3)153x i y i i++-=++，则[ D ] A ．1,11x y =-=- B ．1,11x y =-=C ．1,11x y ==-D ．1,11x y ==3．设()(,)(,)f z u x y iv x y =+在区域D 内解析，则[ B ]A ．()u v f z i x y ∂∂'=+∂∂B ．()u v f z i x x∂∂'=+∂∂ C ．()u v f z i y y ∂∂'=+∂∂ D ．()u v f z i y x ∂∂'=+∂∂ 4．下列说法正确的是[ C ]A ．如果0()f z '存在，则()f z 在0z 处解析B ．如果(,)u x y 和(,)v x y 在区域D 内可微，则()(,)(,)f z u x y iv x y =+在区域D 内解析C ．如果()f z 在区域D 内处处可导，则()f z 在区域D 内解析D ．如果()f z 在区域D 内解析，则()f z 在区域D 内一定不解析5．下列等式中不正确的是[ B ]A ．(1)(21)Ln k i π-=+ （k 为整数）B ．2Lnz Lnz Lnz +=C ．2z k i z e e π+= （k 为整数）D ．22sin cos 1i i +=6．设2222()(2)f z x axy y i bx xy y =+-+++在复平面内处处解析（其中,a b 为常数），则[ C ]A ．2,1a b ==B ．1,2a b ==C ．2,1a b ==-D ．1,2a b =-=7．设Γ为单位圆周1z =，则积分Im zdz Γ⎰的值为[ D ]A ．i πB ．i π-C ．πD ．π-8．级数1!nn n n z n ∞=∑的收敛圆为[ A ] A ．1z e<B ．z e <C ．1z <D ．2e z <9．0z =是函数2()(1)z f z z e =-的[ C ] A ．一级零点 B ．二级零点C ．三级零点D ．四级零点10．设51()sin ,f z z z=则[]Re (),0s f z =[ D ] A ．1 B ．15!C ．1-D ．011.函数2)(z z f =在复平面上 ( C )A.处处不连续B.处处连续，处处不可导C.处处连续，仅在点0=z 处可导D.处处连续，仅在点0=z 处解析12.设复数a 与b 有且仅有一个模为1，则ba b a --1的值 ( B ) A.大于1 B.等于1 C.小于1 D.无穷大13、设x y z f y x z i )(i +-=+=，，则=')(z f ( B )A.i 1+B.iC.1-D.014、设C 是正向圆周 1=z ，i 2sin π=⎰dz z z C n ，则整数n 等于 ( D )A.1-B.0C.1D.215、0=z 是21)(ze zf z -=的 ( A ) A.1阶极点 B.2阶极点 C.可去奇点 D.本性奇点二、填空题（每空4分，共20分）11．Arg = 223k ππ-+ 12．若函数f(z)在整个平面上处处解析，则称它是_____整函数_____。

西北工大复变函数试题

考试题1复变函数 (A 卷)一、填空题（每题4分，共20分）1 12i +=______________2 |z|=21d ()(4)z z i z =+-⎰ 3 幂级数1n n nz ∞=∑的收敛半径R=______________4 1Re [,]sin s z zπ=____________________ 5 函数1zω=将z 平面上的曲线1x =变为ω平面上的（,z x iy u iv ω=+=+）二、单项选择题（每题4分，共20分）.1 设1()sin(1)f z z=-，则0z =是()f z 的【】A ．可去奇点B ．本性奇点C ．极点D ．非孤立奇点.2 设1n > 为正整数，则||21d 1n z z z =-⎰ 为【】 A ．0 B ． 2i π C. i π D. 2n i π3 级数1nn z n∞=∑在||1z =上【】A ．收敛B ．发散C ．既有收敛点也有发散点D ．不确定4 0cos limsin x z z zz z→-=- 【】 A ．3- B. ∞ C. 0 D. 35 设1328()(1)(1)z f z z z =-+，则()f z 在复平面上所有有限奇点处的留数之和等于【】 A ． 1- B. 1 C. 10 D. 0三 (10分) 讨论函数2()f z x iy =-的可微性与解析性。

四 (10分) 设()f z 在||(1)z R R <>内解析，且(0)1f =，(0)2f '=，试计算积分22||1()(1)d z f z z z z =+⎰ 并由此得出22cos ()2i f e d πθθθ⎰之值。

五 (10分) 已知调和函数22(,)u x y x y xy =-+。

求共轭调和函数(,)v x y 及解析函数()(,)(,)f z u x y iv x y =+。

六 (12分) 求函数21()()f z z z i =-在以下圆环域内的Laurent 展式: (1) 0|1z i <-<； (2) 1|z i <-<∞。

复变函数与积分变换A 期末试卷(北京航空航天大学)

2010 —2011 学年第一学期考试统一用答题册题号一二三四五六七八总分成绩阅卷人考试课程复变函数与积分变换A班级学号姓名成绩2011 年 1月 8 日（试题共5页）一、选择题(每题3分，共24分) 1．一个复数乘以i -，则( )（A ）复数的模不变，辐角减少π/2 （B ）复数的模不变，辐角增加π/2。

（C ）复数的模增加，辐角减少π/2。

（D ）复数的模减少，辐角增加π/2。

2．设C 为正向圆周21=z ，则=+---⎰z z z z z C d 10621sin)2(23 ( ) （A ）)1sin 1cos 3(2-i π （B ）0 （C ）1cos 6i π （D ）1sin 2i π- 3．设)(z f 和)(z g 均为整函数，下列命题错误的是（）（A ）)(3z f 是整函数（B ）)()(z g z f 是整函数（C ）)()(z g z f 是整函数（D ）)2(2+z g 是整函数 4. 若,,2,1,3,2,1,0,2⎩⎨⎧--===n n c n n n 则双边幂级数∑+∞-∞=-n n n z c )2(的收敛域为（）（A ）21||31<<z （B ）21|2|31<-<z （C ）3||2<<z （D ）3|2|2<-<z 5．若0z z =是函数)(z f 的m 级零点，则0z 是)(2z f 的( )（A ）m 级零点（B ）m 2级零点（C ）m 级极点（D ）m 2级极点6．设0=z 为函数zz e zsin 1--的m 级极点，那么=m ( )（A ）5 （B ）4 (C)3 （D ）27．∞=z 是函数ziz z ++232的（）（A ）可去奇点（B ）一级极点（C ）二级极点（D ）本性奇点 8. 积分⎰+∞-03d 2sin t t te t 的值为（）（A ）16912(B) 16912- (C) 0 (D) 不存在二、填空题（每题3分，共24分）1．假设21,z z 非零，则||||||||2121z z z z +=+的充分必要条件是21,z z 具有相同的 . 2. 已知一元二次方程求根公式在复数域内仍成立。

《复变函数与积分变换》试卷及答案

得分得分«复变函数与积分变换»期末试题（A ）题号一二三四五六总分得分一．填空题（每小题3分，共计15分）1．231i -的幅角是（）； 2.)1(i Ln +-的主值是（）；3.211)(z z f +=，=)0()5(f （）；4．0=z 是 4sin z zz -的（）极点；5． zz f 1)(=，=∞]),([Re z f s （）；二．选择题（每小题3分，共计15分）1．解析函数),(),()(y x iv y x u z f +=的导函数为（）；（A ） y x iu u z f +=')(；（B ）y x iu u z f -=')(；（C ）y x iv u z f +=')(；（D ）x y iv u z f +=')(.2．C 是正向圆周3=z ，如果函数=)(z f （），则0d )(=⎰Cz z f ．（A ）23-z ；（B ）2)1(3--z z ；（C ）2)2()1(3--z z ；（D ）2)2(3-z . 3．如果级数∑∞=1n nnz c 在2=z 点收敛，则级数在（A ）2-=z 点条件收敛；（B ）i z 2=点绝对收敛；（C ）i z+=1点绝对收敛；（D ）i z 21+=点一定发散．４．下列结论正确的是( )（A ）如果函数)(z f 在0z 点可导，则)(z f 在0z 点一定解析； (B) 如果)(z f 在C 所围成的区域内解析，则0)(=⎰Cdz z f（C ）如果0)(=⎰Cdz z f ，则函数)(z f 在C 所围成的区域内一定解析；（D ）函数),(),()(y x iv y x u z f +=在区域内解析的充分必要条件是),(y x u 、),(y x v 在该区域内均为调和函数．5．下列结论不正确的是（）．(A) 的可去奇点；为z1sin ∞(B) 的本性奇点；为z sin ∞(C) ;1sin 1的孤立奇点为z∞(D) .sin 1的孤立奇点为z ∞ 三．按要求完成下列各题（每小题10分，共计40分）（1）设)()(2222y dxy cx i by axy x z f +++++=是解析函数，求.,,,d c b a得分（2）．计算⎰-Czz z z e d )1(2其中C 是正向圆周：2=z ；（3）计算⎰=++3342215d )2()1(z z z z z（4）函数323 2)(sin)3 ()2)(1()(z zzzzzfπ-+-=在扩充复平面上有什么类型的奇点？，如果有极点，请指出它的级.四、（本题14分）将函数)1(1)(2-=z z z f 在以下区域内展开成罗朗级数；（1）110<-<z ，（2）10<<z ，（3）∞<<z 1得分五．（本题10分）用Laplace 变换求解常微分方程定解问题⎩⎨⎧='==+'-''-1)0()0()(4)(5)(y y e x y x y x y x六、（本题6分）求)()(0>=-ββtet f 的傅立叶变换，并由此证明：te d t ββπωωβω-+∞=+⎰2022cos得分得分«复变函数与积分变换»期末试题（A ）答案及评分标准一．填空题（每小题3分，共计15分）1．231i -的幅角是（ 2,1,0,23±±=+-k k ππ）；2.)1(i Ln +-的主值是（ i 432ln 21π+ ）； 3.211)(z z f +=，=)0()5(f （ 0 ），4．0=z 是 4sin z zz -的（一级）极点；5． zz f 1)(=，=∞]),([Re z f s （-1 ）；二．选择题（每题4分，共24分）1．解析函数),(),()(y x iv y x u z f +=的导函数为（B ）；（A ） y x iu u z f +=')(；（B ）y x iu u z f -=')(；（C ）y x iv u z f +=')(；（D ）x y iv u z f +=')(.2．C 是正向圆周3=z ，如果函数=)(z f （ D ），则0d )(=⎰Cz z f ．（A ）23-z ；（B ）2)1(3--z z ；（C ）2)2()1(3--z z ；（D ）2)2(3-z . 3．如果级数∑∞=1n nnz c 在2=z 点收敛，则级数在（C ）（A ）2-=z 点条件收敛；（B ）i z 2=点绝对收敛；（C ）i z+=1点绝对收敛；（D ）i z 21+=点一定发散．４．下列结论正确的是( B )（A ）如果函数)(z f 在0z 点可导，则)(z f 在0z 点一定解析；(B) 如果)(z f 在C 所围成的区域内解析，则0)(=⎰Cdz z f（C ）如果0)(=⎰Cdz z f ，则函数)(z f 在C 所围成的区域内一定解析；（D ）函数),(),()(y x iv y x u z f +=在区域内解析的充分必要条件是),(y x u 、),(y x v 在该区域内均为调和函数．5．下列结论不正确的是（ D ）．的可去奇点；为、zA 1sin )(∞的本性奇点；为、z B sin )(∞.sin )(的孤立奇点为、zC 11∞的孤立奇点；为、z D sin )(1∞ 三．按要求完成下列各题（每小题10分，共40分）（1）．设)()(2222y dxy cx i by axy x z f +++++=是解析函数，求.,,,d c b a解：因为)(z f 解析，由C-R 条件y v x u ∂∂=∂∂ xv y u ∂∂-=∂∂ y dx ay x 22+=+,22dy cx by ax --=+,2,2==d a ，,2,2d b c a -=-=,1,1-=-=b c给出C-R 条件6分，正确求导给2分，结果正确2分。

(完整版)《复变函数》考试试题与答案各种总结

《复变函数》考试试题（一）一、判断题（20分）:1.若f （z)在z 0的某个邻域内可导，则函数f(z ）在z 0解析. （）2.有界整函数必在整个复平面为常数。

( ) 3。

若}{n z 收敛,则} {Re n z 与}{Im n z 都收敛. ( ）4.若f （z ）在区域D 内解析，且0)('≡z f ，则C z f ≡)(（常数）. ( )5.若函数f （z ）在z 0处解析,则它在该点的某个邻域内可以展开为幂级数. （ ) 6。

若z 0是)(z f 的m 阶零点，则z 0是1/)(z f 的m 阶极点。

( ） 7。

若)(lim 0z f z z →存在且有限，则z 0是函数f （z)的可去奇点. ( )8。

若函数f （z ）在是区域D 内的单叶函数，则)(0)('D z z f ∈∀≠。

（ ) 9. 若f (z )在区域D 内解析, 则对D 内任一简单闭曲线C 0)(=⎰Cdz z f .（）10.若函数f(z ）在区域D 内的某个圆内恒等于常数，则f(z)在区域D 内恒等于常数。

（）二.填空题（20分）1、 =-⎰=-1||00)(z z nz z dz__________.（n 为自然数）2。

=+z z 22cos sin _________. 3.函数z sin 的周期为___________.4.设11)(2+=z z f ，则)(z f 的孤立奇点有__________。

5。

幂级数0n n nz ∞=∑的收敛半径为__________。

6.若函数f （z ）在整个平面上处处解析，则称它是__________。

7。

若ξ=∞→n n z lim ，则=+++∞→n z z z nn (i)21______________.8。

=)0,(Re n zz e s ________，其中n 为自然数。

9. zz sin 的孤立奇点为________ .10。

复变函数历年考试真题试卷

复变函数历年考试真题试卷一、选择题1. 下列哪个函数不是复变函数？A. f(z) = e^zB. f(z) = z^2C. f(z) = |z|D. f(z) = ln(z+1)2. 设f(z) = u(x,y) + iv(x,y)是一个复变函数，下面哪个等式成立？A. ∂u/∂x = ∂v/∂yB. ∂u/∂y = ∂v/∂xC. ∂u/∂x = -∂v/∂yD. ∂u/∂y = -∂v/∂x3. 对于复变函数f(z) = x^3 + 3ix^2y - 3xy^2 - iy^3，下列哪个等式成立？A. ∂u/∂x = 3x^2 + 6ixy - 3y^2B. ∂u/∂y = 3x^2 + 6ixy - 3y^2C. ∂v/∂x = -3x^2 + 3y^2 - 6ixyD. ∂v/∂y = -3x^2 + 3y^2 - 6ixy二、填空题1. 设f(z) = z^2 + 2iz - 1，则f(z)的共轭函数是________。

2. 当z → ∞ 时，f(z) = z^2 + 3z + 1的极限是________。

3. 若f(z) = u(x,y) + iv(x,y) 是全纯函数，则满足柯西-黎曼方程的条件是∂u/∂x = ________。

三、计算题1. 计算复变函数f(z) = z^3 - 4z的积分，其中C为以原点为圆心、半径为2的圆周。

2. 当z = -i 时，计算复变函数f(z) = 2z^2 + 3iz的导数。

四、证明题证明：若复变函数f(z) = u(x,y) + iv(x,y) 在单连通域D上解析，则f(z) 在D 上也是调和函数。

（请自行根据题目要求增减字数，使得文章达到合适的长度。

）（文章正文）选择题：1. 下列哪个函数不是复变函数？2. 设f(z) = u(x,y) + iv(x,y)是一个复变函数，下面哪个等式成立？3. 对于复变函数f(z) = x^3 + 3ix^2y - 3xy^2 - iy^3，下列哪个等式成立？填空题：1. 设f(z) = z^2 + 2iz - 1，则f(z)的共轭函数是________。

复变函数考试试题

复变函数考试试题复变函数考试试题一、选择题1. 下列哪个函数是复变函数？A. f(x) = 3x^2 + 2x + 1B. f(z) = z^2 + 1C. f(x) = e^xD. f(z) = |z|2. 设函数f(z) = u(x, y) + iv(x, y)，其中u(x, y)和v(x, y)为实函数，z = x + iy，那么下列哪个条件是复变函数的充分条件？A. u(x, y)和v(x, y)都满足柯西-黎曼方程B. u(x, y)和v(x, y)都是连续函数C. u(x, y)和v(x, y)都是可微分函数D. u(x, y)和v(x, y)都是可积函数3. 设f(z) = z^2 + 1，那么f(z)的共轭函数为：A. f(z) = z^2 - 1B. f(z) = z^2 + 1C. f(z) = z^2 - iD. f(z) = z^2 + i4. 设f(z) = e^z，那么f(z)的实部和虚部分别是：A. 实部：e^x，虚部：e^yB. 实部：cos(x)，虚部：sin(y)C. 实部：e^x，虚部：sin(y)D. 实部：cos(x)，虚部：e^y二、填空题1. 设f(z) = z^3，那么f(z)的导数为_________。

2. 设f(z) = e^z，那么f(z)的导数为_________。

3. 设f(z) = z^2 + 1，那么f(z)的积分为_________。

三、解答题1. 证明：若函数f(z)在某个区域D内解析，则f(z)在D内连续。

2. 设函数f(z) = u(x, y) + iv(x, y)在区域D内解析，且满足柯西-黎曼方程，即u_x = v_y，u_y = -v_x。

证明：函数g(z) = u(x, y) - iv(x, y)也在区域D内解析。

3. 设函数f(z) = z^2 + z + 1，在区域D内解析。

求出D上的一个原函数F(z)，并计算∮_C f(z)dz，其中C为D内的任意简单闭合曲线。

答案复变函数A

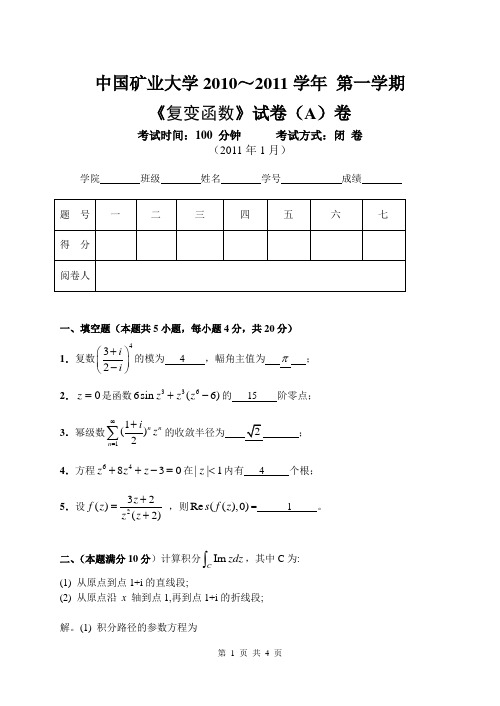
中国矿业大学2010～2011学年第一学期《复变函数》试卷（A ）卷考试时间：100 分钟考试方式：闭卷（2011年1月）学院班级姓名学号成绩一、填空题（本题共5小题，每小题4分，共20分）1．复数432+⎛⎫⎪-⎝⎭i i 的模为 4 ，輻角主值为 π ；2．0=z 是函数3366sin (6)+-z z z 的 15 阶零点；3．幂级数11()2∞=+∑n n n i z4．方程64830++-=z z z 在1||<z 内有 4 个根； 5．设232()(2)+=+z f z z z ，则Re ((),0)s f z = 1 。

二、（本题满分10分）计算积分Im ⎰Czdz ，其中C 为:(1) 从原点到点1+i 的直线段;(2) 从原点沿 x 轴到点1,再到点1+i 的折线段;解。

(1) 积分路径的参数方程为it t t z +=)(， )10(≤≤t …………………………………………….1’于是Im ⎰Czdz =11(1)2it i dt ++=⎰……………………………………………4’ (2) 积分路径由两段直线段构成x 轴上直线段的参数方程为t t z =)(， )10(≤≤t1到1+i 直线段的参数方程为it t z +=1)(， )10(≤≤t ………………1’ 于是Im ⎰Czdz =12=⎰iitdt ……………………………………………………...4’ 三、（本题满分15分）计算积分2(1)-⎰zC e dz z z ，其中C 为圆周||3=z .解：||3=z 内有奇点0,1, 1.=-z ……………………………….6’ 由Cauchy 公式2(1)-⎰zC e dz z z =-⎰z C e dz z +121-⎰z C e dz z +121+⎰z C e dz z ………….3’=02π=-z z ie+1π=z z ie+1π=-z z ie……………….3’=1(2)π-+-i e e …………………………………..3’ 四、（本题满分10分）计算积分3cos ⎰C zdz z ，其中C 为绕0=z 的正向闭曲线。

复变函数考试试卷(A)及答案

第 1 页共 5 页考试试卷(A)2008--2009学年第二学期时间110分钟复变函数与积分变换课程40学时2.5学分考试形式: 闭卷一、专业年级: 教改信息班总分100分, 占总评成绩70 %1．注: 此页不作答题纸, 请将答案写在答题纸上单项选择题（15分, 每小题3分）下列方程中, 表示直线的是（）。

()()()()()()()254(54)54(54)112Re 1A i z i z zzB i z i zC z i z iD z z z -++=-++=-++==-2．函数222()()(2)f z x y x i xy y =--+-在（）处可导。

()()()()22A B x C y D ==全平面处处不可导下列命题中, 不正确的是（）。

()()()()()()()()()0Res ,0Im 1.z z A f z f z B f z D z f z D C e iD z e iωπω∞∞=-=<<<+如果无穷远点是的可去奇点,那么若在区域内任一点的邻域内展开成泰勒级数,则在内解析.幂级数的和函数在收敛圆内是解析函数.函数将带形域0()映射为单位圆3．下列级数绝对收敛的是（）。

()()()()()221111112n nnn n n n i i i A B C i D nnn ∞∞∞∞====⎛⎫++⎪⎝⎭∑∑∑∑ 设在内解析且 , 那么（）。

()()()()2211A iB iCD ππ--第 2 页共 5 页1. 的主值为。

2. 函数仅在点z= 处可导。

3. 。

4. 函数在处的泰勒展开式。

5. 幂级数的收敛半径为。

三．(10分)求解析函数 , 已知。

四. (20分)求下列积分的值 1．()2241z z e dz zz =-⎰2．()20sin 0x xdx a x a+∞>+⎰五. (15分)若函数在点解析, 试分析在下列情形: 1. 为函数的m 阶零点； 2. 为函数的m 阶极点；求()()()0Res ,f z z z f z ϕ⎡⎤'⎢⎥⎣⎦。

复变函数练习题

复变函数练习题1. 求下列复变函数的导数：a) $f(z) = z^3 - 2z^2 + 4z - 3$b) $g(z) = e^z \sin(z)$c) $h(z) = \frac{1}{z^2+1}$2. 计算下列复变函数的积分：a) $\int_C (3z^2 - 2\bar{z}) \, dz$，其中 $C$ 是由圆 $|z|=2$ 给出的路径。

b) $\int_C \cos(z) \, dz$，其中 $C$ 是由直线段 $z=1$ 到 $z=i$ 给出的路径。

c) $\int_C \frac{1}{z^2-4} \, dz$，其中 $C$ 是由两个阶梯型路径组成的，从 $z=-2$ 到 $z=-1$，然后从 $z=-1$ 到 $z=2$。

3. 求下列复变函数的奇点，并判断其类型（可去奇点、极点或本性奇点）：a) $f(z) = \frac{1}{z^2+1}$b) $g(z) = \frac{\sin(z)}{z}$c) $h(z) = \frac{1}{\sqrt{z+2}}$4. 计算下列复变函数的Laurent级数展开：a) $f(z) = \frac{1}{z^2(z-1)}$b) $g(z) = \frac{e^z}{z^3}$c) $h(z) = \frac{1}{(z^2-1)^2}$5. 利用残数定理计算下列积分：a) $\int_C \frac{e^z}{z(z-1)^3} \, dz$，其中 $C$ 是由圆 $|z|=2$ 给出的路径。

b) $\int_C \frac{\ln(z)}{z(z+1)} \, dz$，其中 $C$ 是由圆 $|z-1|=1$ 给出的路径。

c) $\int_C \frac{1}{e^z-1} \, dz$，其中 $C$ 是由直线段 $z=-\pi$ 到$z=\pi$ 给出的路径。

以上是关于复变函数练习题的内容，通过解答这些问题，可以加深对复变函数的理解。

《复变函数》期末试卷及答案(A卷)

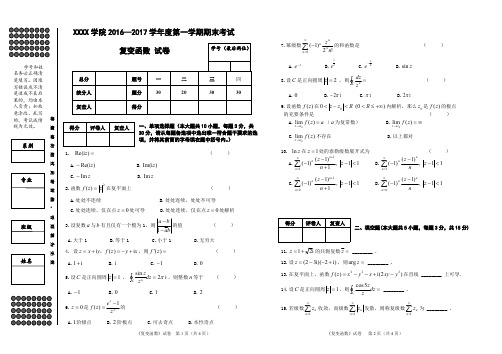
20.计算积分 1i z 2dz . 0
17.判断数列 zn

2017 ni n 1
的收敛性.
若收敛，求出其极限.
18.求在映射 w z2 下， z 平面上的直线 z (2 i)t 被映射成 w 平面上的曲线的方程.
得分
评卷人复查人
三、证明题(本大题共 1 小题，每小题 15 分，共 15 分)
二
题分
30
20
得分
三
四
30
30
得分
评卷人复查人
一、单项选择题（本大题共 10 小题，每题 3 分，共 30 分，请从每题备选项中选出唯一符合题干要求的选项，并将其前面的字母填在题中括号内。）
1. Re(iz)
A. Re(iz)
B. Im(iz)
C. Im z
D. Im z
2.函数 f (z) z 2 在复平面上
21.试证明柯西不等式定理:设函数 f (z) 在圆 C : z z0 R 所围的区域内解析，且在
C 上连续，则
f
(n) (z0 )

Mn! Rn
(n 1,2,...)
其中 M 是 f (z) 在 C 上的最大值.
19.求 ez 在 z 0 处的泰勒展开式.
《复变函数》试卷第 3 页（共 4 页）
这是 w 平面上第一象限内的一条半直线。
19. 解：因为 (ez )(n) ez (n 0,1,2,...) ，其展开式中泰勒系数为
cn

f (n) (0) n!

1 n!
于是 ez 在 z 0 处的泰勒展开式为
ez zn 1 z zn zn

复变函数试题及答案

复变函数试题及答案一、选择题（每题4分，共40分）1. 下列哪个函数在全平面上是解析的？A. f(z) = |z|^2B. f(z) = e^zC. f(z) = ln(z)D. f(z) = 1/z答案：B2. 设f(z) = u(x, y) + iv(x, y)是解析函数，其中u(x, y)和v(x, y)是实函数。

下列哪个条件是解析函数的充分必要条件？A. u满足柯西-黎曼方程B. v满足柯西-黎曼方程C. u和v满足柯西-黎曼方程D. u和v的一阶偏导数满足柯西-黎曼方程答案：C3. 设f(z) = u(r, θ)是解析函数，其中r和θ是极坐标系下的变量。

下列哪个条件是解析函数的充分必要条件？A. u满足极坐标下的柯西-黎曼方程B. f(z)在全平面上是解析的C. f(z)在圆心附近是解析的D. f(z)在正实轴上是解析的答案：A4. 设f(z) = u(x, y) + iv(x, y)是解析函数，其中u(x, y)和v(x, y)是实函数。

若u和v满足柯西-黎曼方程，则A. f(z)在全平面上是解析的B. f(z)在实轴上是解析的C. f(z)在虚轴上是解析的D. f(z)在解析的那部分上满足柯西-黎曼方程答案：A5. 设f(z) = u(x, y) + iv(x, y)是解析函数，其中u(x, y)和v(x, y)是实函数。

若f(z)在实轴上是解析的，则A. u(x, y)在全平面上是解析的B. v(x, y)在全平面上是解析的C. u(x, y)和v(x, y)满足柯西-黎曼方程D. u(x, y)和v(x, y)处处可微分答案：C二、填空题（每空5分，共30分）1. 若f(z) = x^2 - y^2 + 2xyi是解析函数，则它的共轭函数为________。

答案：f*(z) = x^2 - y^2 - 2xyi2. 设f(z) = u(x, y)是解析函数，且满足柯西-黎曼方程的实部形式，则函数f(z)可表示为f(z) = ________。

复变函数复习资料精选全文完整版

可编辑修改精选全文完整版复变函数论（A ）Ⅰ. Cloze Tests （20102=⨯ Points ）1. If nnn n i i z ⎪⎭⎫⎝⎛++⎪⎭⎫ ⎝⎛-=1173，thenlim =+∞→n n z .2. If C denotes the circle centered at 0z positively oriented and n is apositive integer ，then)(10=-⎰C n dz z z . 3. The radius of convergence of∑∞=++13)123(n n z n nis .4. The singular points of the function )3(cos )(22+=z z zz f are . 5. 0 ,)ex p(s Re 2=⎪⎭⎫⎝⎛n z z , where n is a positive integer.6.=)sin (3z e dzd z. 7. The main argument and the modulus of the number i -1 are . 8. The square roots of i -1 are . 9. The definition of z e is . 10. Log )1(i -= .Ⅱ. True or False Questions (1553=⨯ Points)1. If a function f is analytic at a point 0z ，then it is differentiable at 0z .（）2. If a point 0z is a pole of order k of f ，then 0z is a zero of order k off /1.（）3. A bounded entire function must be a constant.（）4. A function f is analytic a point 000iy x z += if and only if whose real andimaginary parts are differentiable at ),(00y x .（）5. If f is continuous on the plane and =+⎰Cdz z f z ))((cos 0 for every simpleclosed path C , then z e z f z 4sin )(+ is an entire function. ( )Ⅲ. Computations (3557=⨯ Points)1. Find⎰=-+1||)2)(12(5z z z zdz.2. Find the value of ⎰⎰==-+228122)1(sin z z z z dzz dz z ze . 3. Let )2)(1()(--=z z zz f ，find the Laurent expansion off on the annulus{}1||0:<<=z z D .4. Given λλλλd z z f C⎰-++=345)(2，where {}3|:|==z z C ，find )1(i f +-'.5. Given )1)(1(sin 1)(2+-+=z z zz f ，find )1),(Res()1),(Res(-+z f z f .Ⅳ. Verifications (30310=⨯ Points)1. Show that if )(0)()(C z z f k ∈∀≡, then )(z f is a polynomial of order k <.2. Show that 012797lim 242=+++⎰+∞→R C R dz z z z , where R C is the circle centered at 0 with radius R .3. Show that the equation 012524=-+-z z z has just two roots in the unite disk复变函数论（B ）Ⅰ. Cloze Tests （20102=⨯ Points ）1. If nnn n i i z ⎪⎭⎫⎝⎛++⎪⎭⎫ ⎝⎛-=1162，thenlim =+∞→n n z .2. If C denotes the circle centered at 0z positively oriented and n is apositive integer ，then)(10=-⎰C n dz z z . 3. The radius of the power series∑∞=+12)1(n n z nis .4. The singular points of the function )1(sin )(2+=z z zz f are .5. 0 ,)ex p(s Re 2=⎪⎭⎫⎝⎛n z z , where n is a positive integer.6.=z e dzd z2cos . 7. The main argument and the modulus of the number i -1 are . 8. The square roots of 1+i are . 9. The definition of z cos is . 10. Log )1(i += .Ⅱ. True or False Questions (1553=⨯ Points)1. If a function f is differentiable at a point 0z ，then it is continuous at 0z .（）2. If a point 0z is a pole of order m of f ，then 0z is a zero of order m off /1.（）3. An entire function which maps the plane into the unite disk must be aconstant.（）4. A function f is differentiable at a point 000iy x z += if and only if whosereal and imaginary parts are differentiable at ),(00y x and the Cauchy Riemann conditions hold there.（）5. If a function f is continuous on the plane and=⎰Cdz z f )(0 for everysimple closed contour C , then z z f sin )( is an entire function. ( )Ⅲ. Computations (3557=⨯ Points)1. Find⎰=-+1||)2)(12(z z z zdz.2. Find the value of ⎰⎰==-+223122)1(sin z z z z dzz dz z ze . 3. Let )2)(1()(--=z z zz f ，find the Laurent expansion off on the annulus{}1||0:<<=z z D .4. Given λλλλd z z f C⎰-++=142)(2，where {}3|:|==z z C ，find )1(i f +-'.5. Given )1)(1(sin )(2+-=z z zz f ，find )1),(Res()1),(Res(-+z f z f .Ⅳ. Verifications (30310=⨯ Points)1. Show that the function iy x e e z z f ---=)2()(2is an entire function.2. Show that if )(0)()(C z z f m ∈∀≡, then )(z f is a polynomial of orderm <.3. Show that 0651lim 242=+++⎰+∞→R C R dz z z z , where R C is the circle centered at 0 with radius R .复变函数论（C ）Ⅰ. Cloze Tests （20102=⨯ Points ）1. If nn n n i i z ⎪⎭⎫⎝⎛++⎪⎭⎫ ⎝⎛+=3131，thenlim =+∞→n n z .2. If C denotes any simple closed contour and 0z is a point inside C , then)(sin 0=-⎰Cn dz z z z, where n is an integer. 3. The radius of convergence of the power series∑∞=-12)63(n n z nis .4. The singular points of the function )2(cos )(244-+=z z z z z f are .5. 0 ,)ex p(s Re =⎪⎭⎫⎝⎛m z z , where m is a positive integer.6. The main argument and the modulus of the number iie 45πare . 7. The integral of the function )(sin )(2ti t t t w += on ]1,1[- is . 8. The definition of z sin is . 9. Log )1(i -= .10. The solutions of the equation 013=-zi e are .Ⅱ. True or False Questions (1553=⨯ Points)1. If a function f is continuous at a point 0z ，thenit is differentiable at 0z .（）2. If a point 0z is a pole of order m of f ，then there is a function ϕ that isanalytic at 0z with 0)(0≠z ϕ such that mz z z z f )()()(0-=ϕ on somedeleted neighborhood of 0z .（）3. An entire function which is identically zero on a line segment must beidentically zero.（）4. A function f is differentiable on open set D if and only if whose real andimaginary parts are differentiable on D and the Cauchy Riemann conditions hold on D .（）5. If a function f is continuous on the plane and=⎰Cdz z f )(0 for everysimple closed path C , then 0)(=z f for all z . ( )Ⅲ. Computations (3557=⨯ Points)1. Find⎰=++1||)23)(13(9z z z zdz.2. Find the value of ⎰⎰==-+-222142)1(sin z z z dzz dz z zz . 3. Let )2)(1(3)(2++=z z z z f ，find the Laurent expansion of f on the annulus{}1||0:<<=z z D .4. Given ξξξξd z z f C ⎰-++=543)(2，where {}4|:|==z z C ，find )2(i f +'.5. Find ⎪⎪⎭⎫⎛+i z z ,)1(4Res 222.Ⅳ. Verifications (30310=⨯ Points)1. Show that 0233lim 242=+++⎰+∞→RC R dz z z z , where R C is the circle centered at 0 with radius R .2. Suppose that f is analytic and ||f is a constant on a domain a domainD , prove that a z f =)( for some constant a and all D z ∈.3. Show that the equation z z z z -=+-127234 has just three roots in the unite disk.《复变函数论》试题（D ）Ⅰ. Cloze Tests （20102=⨯ Points ）1. If nnn n i i z ⎪⎭⎫⎝⎛++⎪⎭⎫ ⎝⎛-=1153，then lim =+∞→n n z .2. If C denotes the circle centered at 0z positively oriented and n is apositive integer ，then)(10=-⎰Cn dz z z . 3. The radius of the power series∑∞=++13)12(n n z n nis .4. The singular points of the function )3(cos )(2+=z z zz f are . 5. 0 ,)ex p(s Re 2=⎪⎭⎫⎝⎛n z z , where n is a positive integer.6.=)sin (5z e dzd z. 7. The main argument and the modulus of the number i -1 are . 8. The square roots of 1+i are . 9. The definition of z e is . 10. Log )1(i += .Ⅱ. True or False Questions (1553=⨯ Points)1. If a function f is differentiable at a point 0z ，then it is analytic at 0z .（）2. If a point 0z is a pole of order k of f ，then 0z is a zero of order k off /1.（）3. A bounded entire function must be a constant.（）4. A function f is analytic a point 000iy x z += if and only if whose real andimaginary parts are differentiable and the Cauchy Riemann conditions hold in a neighborhood of ),(00y x .（）5. If a function f is continuous on the plane and=⎰Cdz z f )(0 for everysimple closed contour C , then z e z f z sin )(+ is an entire function. ( )Ⅲ. Computations (3557=⨯ Points)1. Find⎰=-+1||)2)(12(z z z zdz.2. Find the value of ⎰⎰==-+223122)1(sin z z z z dzz dz z ze . 3. Let )2)(1()(--=z z zz f ，find the Laurent expansion off on the annulus{}1||0:<<=z z D .4. Given λλλλd z z f C⎰-++=142)(2，where {}3|:|==z z C ，find )1(i f +-'.5. Given )1)(1(sin )(2+-=z z zz f ，find )1),(Res()1),(Res(-+z f z f .Ⅳ. Proving (30310=⨯ Points)1. Show that if )(0)()(C z z f m ∈∀≡, then )(z f is a polynomial of order m <.2. Show that 012783lim 242=+++⎰+∞→R C R dz z z z , where R C is the circle centered at 0 with radius R .3. Show that the equation 012524=-+-z z z has just two roots in the unitedisk.《复变函数论》试题（E ）Ⅰ. Cloze Tests （20102=⨯ Points ）1. If nn n i n n z ⎪⎭⎫⎝⎛++-=211，thenlim =+∞→n n z . 2. If C denotes the circle centered at 0z and n is an integer ，then)(1210=-⎰C n dz z z i π. 3. The radius of the power series∑∞=+12)1(n n z nis .4. The singular points of the function 1cos )(2+=z zz f are .5. 0 ,sin s Re 2=⎪⎭⎫⎝⎛n z z , where n is a positive integer.6.=z e dzd z2sin . 7. The main argument and the modulus of the number i +1 are . 8. The square roots of )0(>A Ai are . 9. The definition of z cos is . 10. Log )22(i += .Ⅱ. True or False Questions (1553=⨯ Points)1. If a function f is differentiable at a point 0z ，then it is continuous at 0z .（）2. If a point 0z is a zero of order n of f ，then 0z is a pole of order n off /1.（）3. There is a non-constant entire function which maps the plane into the disk1000||<z .（）4. A function f is differentiable at a point 000iy x z += if and only if whosereal and imaginary parts are differentiable at ),(00y x and the Cauchy Riemann conditions hold there.（）5. If a function f is continuous on the plane and=⎰Cdz z f )(0 for everysimple closed contour C , then it is an entire function. ( )Ⅲ. Computations (3557=⨯ Points)1. Find the integral ⎰+Czdz z e 12, where C is the circle 7||=z .2. Find the value of ⎰⎰==+-+235121)1(sin z z z z dzz dz z ze . 3. Let )2)(1(1)(--=z z z f ，find the Laurent expansion off on the annulus{}1||0:<<=z z D .4. Given λλλλd z z f C ⎰-++=765)(2，where {}4|:|==z z C ，find )1(i f +'.5. Given )0(2:,2)(πθθ≤≤=+=i e z C zz z f ，find dz z f C⎰)(.Ⅳ. Proving (30310=⨯ Points)1. Show that 020914lim 242=++-⎰+∞→R C R dz z z z , where R C is the circle centered at 0 with radius R .2. Suppose that f is an entire function and there is a constant M and apositive integer m such that )(|||)(|C ∈∀≤z z M z f m . Prove thatm m z a z a z a z f +++= 221)(for some constants 1a , m a a ,,2 and all z in the plane.3·Show that the equation 01438=-+-z z z has just three roots in the unite disk2005－2006学年第一学期期末考试2003级数学与应用数学专业《复变函数论》试题（C ）Ⅰ. Cloze Tests （20102=⨯ Points ）1. If nnn n i i z ⎪⎭⎫⎝⎛++⎪⎭⎫ ⎝⎛+=2121，then lim =+∞→n n z . 2. If C denotes any simple closed contour and 0z is a point inside C , then)(10=-⎰Cn dz z z , where n is an integer. 3. The radius of the power series∑∞=123n n z nis .4. The singular points of the function )2(cos )(24-=z z zz f are .5. 0 ,)ex p(s Re =⎪⎭⎫⎝⎛nz z , where n is a positive integer.6. The main argument and the modulus of the number iie 42π are . 7. The integral of the function )(sin )(4i t t t w += on ]1,1[- is . 8. The definition of z cos is . 9. Log )1(i -= .10. The solutions of the equation 012=-zi e are .Ⅱ. True or False Questions (1553=⨯ Points)1. If a function f is continuous at a point 0z ，then it is differentiable at 0z .（）2. If a point 0z is a pole of order m of f ，then there is analytic function ϕat 0z with 0)(0≠z ϕ such that mz z z z f )()()(0-=ϕ on some deletedneighborhood of 0z .（）3. An entire function which is identically zero on the real axis must be zero.（）4. A function f is differentiable on a domain D if and only if whose realand imaginary parts are differentiable on D and the Cauchy Riemann conditions hold on D .（）5. If a function f is continuous on the plane and=⎰Cdz z f )(0 for everysimple closed contour C , then 0)(=z f for all z . ( )Ⅲ. Computations (3557=⨯ Points)1. Find⎰=++1||)23)(13(z z z zdz.2. Find the value of ⎰⎰==-+-22216)1(sin z z z dzz dz z zz . 3. Let )2)(1()(2++=z z z z f ，find the Laurent expansion of f on the annulus{}1||0:<<=z z D .4. Given ξξξξd z z f C⎰-++=143)(2，where {}4|:|==z z C ，find )2(i f +'.5. Evaluate ),)1((Res 222i z z +. Ⅳ. Proving (30310=⨯ Points)1. Show that 02316lim 242=+++⎰+∞→R C R dz z z z , where R C is the circle centered at 0 with radius R .2. Suppose that f is differentiable and ||f is a constant on a domain D ,prove that A z f =)( for some constant A and all D z ∈.3. Show that the equation 0127234=-++-z z z z has just three roots in theunite disk.复变函数考试试题（G ）1. 求通过1z 和2z 的线段的参数方程（用复数形式表示）。