概率论英文翻译试卷

合集下载

上外贤达学院概率论与数理统计第一学期(英文版)试卷-推荐下载

3.Let the distribution function of the random variable X be
F
(
x)

A
0,
A and B are equal to ( )
设随机变量 X 的分布函数为
F
(
x)

A
0,

Be

Be
1 2
1 2
x2
x2
,
x0
,
3.(15%)A machine produces defective parts with three different probabilities
depending on its state of repair. If the machine is in good working order,it produces defective parts with probability 0.02. If it is wearing down, it produces defective parts with probability 0.1. If it needs maintenance, it produces defective parts with probability 0.3. The probability that the machine is in good working order is 0.8, the probability that it is wearing down is 0 .1, and the probability that it needs maintenance is 0.1. Compute the probability that a randomly selected part will be defective. 一台机器生产不合格部件的概率与机器所处的状态有关。如果机器处于比较好的工作状态，则该概率为 0.02；如果机器处于磨损状态，则该概率为 0.1；如果机器需要维修，则该概率为 0.3. 而机器处于比较好的工作状态的概率为 0.8，处于磨损状态的概率为 0.1，处于需要维修状态的概率为 0.1.求随机选择一个部件，该部件为不合格品的概率。 4 (10%)Two students A and B are both registered for a certain course. Assume that student A attends class 80 percent of the time, student B attends class 60 percent of the time, and the absences of the two students are independent. a What is the probability that at least one of the two students will be in class on a given day? b If at least one of the two students is in class on a given day, what is the probability that A is in class that day? 两个学生 A 和 B 都选了同一门课。假定学生 A 的出勤率为 80%，学生 B 的出勤率为 60%，两个学生是否出勤是相互独立的。求（a）某一天至少有一个学生出勤的概率；（b）假定某一天至少有一个学生出勤，求出勤的学生是 A 的概率。

概率论与数理统计英文题目

Test1. Consider the set {}9,8,7,6,5,4,3,2,1=Ω with subsets {}{}{}{}8,7,4,3,2,1,8,6,4,2,9,7,5,3,1====D C B AFind the following sets:(a) D A and B A (b) c D C )( and )(D B A2. ShowDe Morgans Law: (a) cc c F E F E =)(,(b)c c c F E F E =)(3.(a)Let P(A)=,P(B)=,P( A∩B)=. Find P(A∪B) and P(A|B)(b)Consider two fair dice A and B. Die A is six-sided and is numbered 1 through to 6 whilst die B is four-sided and is numbered 1 through 4. Both dice are rolled. Find the probability of two dice show the same score. 4.(a).Let X be a random variable. ),(~2σμN X . Show that)1,0(~N X Y σμ-=(b)The pmf of a random variable X which has a Poisson distribution with parameter 2. Find P(X=3).5. Suppose (X,Y) be bivariate normal distribution.);,,,(~),(222211ρσμσμN Y X . Find the correlation coefficient of Xand Y.6. (a)Let X Uniformly distributed on (-1,1), . X~U(-1,1). Find the Expectation and Variance of X.(b)Let X and Y be continuous random variables with joint pdf.),(),(2,R y x y x f Y X ∈ Let Z=X+Y, Find )(z f Y X +. In particular, if Xand Y are independent, Find f X *f Y..7.(a)The random variable X has pmf is: X 0 1 2 3 P X (x)Find P(1≤X<3) and F X .(b)Let X be a continuous random variable with pdf⎩⎨⎧≥=-otherwise x e x f x 02)(2 Find P(X=3), P(X>1) and )12(>>X X P . 8. X is continuous . with pdf22102()102xxe xf x e x -⎧≤⎪⎪=⎨⎪>⎪⎩Let X Y =, Find E(Y)X be a continuous random variable with probability density function⎩⎨⎧>=-otherwise x xe x f x 0)( (a) Find P(1<X<2) (b) Find: (1) E(X); ,(2) Given that E(X 2)=6. Find Var(X)10．If the joint probability density function of two random variables is given by ⎩⎨⎧>>=--otherwise y x e x f y x 00,06)(32(a) Find the marginal pdf of X and Y.(b)Determine whether the two random variables are dependent or independent.11．Suppose the random variable X has the density function⎪⎩⎪⎨⎧>+=otherwise x x x f 00)4(32)(3Find the probability density function of the random variable Y=X+4. 12. Take out a number from 1-200 ( 200 natural numbers).Find (a) the probability that this number can be drived by 6;(b) the probability that this number can be drived by 6 as well as 8.13. Three children are selected at random from a group of five boys and three girls.(a)What is the probability that all three are boys?(b)What is the probability at least two girls are selected?14. (a)Let ).(,)(),()(B findP p A P B A P AB P ＝= (b)Let 51)(=A P ，32)|(=B A P ，53)|(=A B P .Find )(B P . 15.(a) Suppose A, B are two events, and P(A)=1/4, P(B)=1/2, P(AB)=1/9, then evaluate ()P AB(b)Use Bayes’ Theorem to show that if P(A),P(B)>0 and )|()(B A p A p < then )()(A B P B P <16.(a)Let X has a Binomial distribution with parameters n and p, . X ~ b (n, p).Find )2(=X P(b) Show that )()()(),(Y E X E XY E Y X Cov -=17. Suppose the distribution function of a random variable X is20,0(),011,1x F x x x x <⎧⎪=≤≤⎨⎪>⎩，Find (a) the probability that X gets value within ，;(b) the density function of X18. The operational lifetime X, in years, of a battery powered watch has probability density function⎩⎨⎧≤≤-=otherwise x x cx x f 063)6()( (a) Find the value of c.(b) Find the cumulative distribution function of X.(c) Find the probability that the watch has an operational lifetime in excess of 4 years.Find a and (3.2)X F(b) A continuous random variable X having the probability density function 212()30x x f x elsewhere⎧-<<⎪=⎨⎪⎩.Find ()f x dx +∞-∞⎰ and P(0<X<1).20.(a)Let )(31)(),()(B P A P A B P A B P ===.FindP(AB) (b)SupposeXandYare independent random variables),3(~),,2(~p B Y p B X ,and .95)1(=≥Y P Find ).1(≥Y P21. Suppose the density function of (X,Y) is()()6,02,24,0,k x y x y f x y --<<<<⎧⎪=⎨⎪⎩else (a) Determine the constant k. (b) Find the probability P{X<1,Y<3}.22. Let X denote the number of times a certain numerical control machinewill malfunction: 1,2,or 3 times on any given day. Let Y denote the number of times a technician is called on an emergency call. Their joint probability distribution is given as Table TableXY 1 2 3 1 23 0(a) Evaluate the marginal distribution of X and Y(b) Determine whether the two random variables of X and Y are dependent or independent.23.(a) If 2~(20.4)X N -，, then find 2(3)E X + (b) Let (X,Y) be 2-dimensional random variables,and(,)(1,0)(1,1)(2,0)(2,1)0.40.2X Y P a bIf ()0.8E XY =，find Cov(,)X Y .the probability distribution of i X is as follows,and 1)0(21==X X P . Find the correlation coefficient of X and Y.25. Let X has a be uniform distribution on the interval (0,1),and 142+-=X X Y Find (a))(y f Y (b) E(Y)26. The random variable X, for fixed 0<p<1 and n ≥1,has probability mass function (pmf)⎩⎨⎧==otherwisen k cp x p kx 0,...,2,1)( (a)Find c in terms of p and n.(b)Find the cumulative distribution function of X.27. If ~[0,1]X U ，~[0,1]Y U ,X and Y are independent. Find ||E X Y -.28. The length of time, in minutes, for an airplane to obtain clearance for take off at a certain airport is a random variable Y=3X-2, where X has the density function⎪⎩⎪⎨⎧>=-.,00,41)(4/elsewhere x ex f xFind the mean and variance of the random variable Y.29. Suppose the probability density function of random variable X is1(1)19()320..x x f x o w ⎧-<<⎪=⎨⎪⎩,and 1(1)2Y X =-.Find (a) the probability density function of Y,)(y f Y .(b) expectation E(Y) and variance Var(Y).30. Suppose X and Y are two random variables. The joint probability density function is⎩⎨⎧≤≤≤≤=otherwisey x xyy x f 010,104),(Find (a) the marginal density of X.(b) expectation E(Y) and variance Var(Y).(c)Determine whether the two random variables are dependent or independent ？。

概率英文测试

The UnivΒιβλιοθήκη rsity of Melbourne
Semester 1 Assessment — June, 2005 Department of Mathematics and Statistics 620-201 Probability
Exam Duration: 3 Hours Reading Time: 15 Minutes This paper has 5 pages
d
d
5. (a) Consider independent discrete random variables X and Y with probability mass functions pX (x) and pY (y ) respectively. Let SX be the set of possible values of X . Using the Law of Total Probability and justifying all your steps, derive the expression pX (x)pY (z − x) pZ (z ) =
6. Let X and Y have joint probability density function f(X,Y ) (x, y ) = kx 0 < x < y < 1 0 elsewhere.
(a) Find k . (b) Explain why X and Y are dependent (no calculations should be required). (c) Find the marginal probability density functions of X and Y . (d) For a ﬁxed y ∈ (0, 1) write down the set of values of x for which fX |Y =y (x|y ) is non-zero. (e) Find fX |Y =y (x|y ) over the range that you derived in (d). (f) Find E (X |Y ).

a first course in probility

SIAM J.N UMER.A NAL .c2010Society for Industrial and Applied Mathematics Vol.48,No.3,pp.1136–1162MEAN FIELD GAMES:NUMERICAL METHODS ∗YVES ACHDOU †AND ITALO CAPUZZO-DOLCETTA ‡Abstract.Mean ﬁeld type models describing the limiting behavior,as the number of players tends to +∞,of stochastic diﬀerential game problems,have been recently introduced by sry and P.-L.Lions [C.R.Math.Acad.Sci.Paris ,343(2006),pp.619–625;C.R.Math.Acad.Sci.Paris ,343(2006),pp.679–684;Jpn.J.Math.,2(2007),pp.229–260].Numerical methods for the approx-imation of the stationary and evolutive versions of such models are proposed here.In particular,existence and uniqueness properties as well as bounds for the solutions of the discrete schemes are investigated.Numerical experiments are carried out.Key words.mean ﬁeld games,ﬁnite diﬀerence schemesAMS subject classiﬁcations.65M06,65M012,91-08,91A23,49L25DOI.10.1137/0907584771.Introduction.Mean ﬁeld type models describing the limiting behavior of stochastic diﬀerential game problems as the number of players tends to +∞have recently been introduced by sry and P.-L.Lions [11,12,13].In the stationary setting,a typical model of this kind comprises the following system:−νΔu +H (x,∇u )+λ=V [m ]in T 2,(1)−νΔm −div m ∂H ∂p (x,∇u ) =0in T 2,(2)T 2u =0, T 2m =1,m >0.(3)The unknowns are the scalar functions u ,m deﬁned on the two-dimensional torus T 2and the real number λ.We consider bidimensional problems for the sake of simplicity,but the results below hold for any space dimension.The data are a positive number ν,the Hamiltonian H :T 2×R 2→R ,convex with respect to p and the (nonlinear)mapping V associating to a probability density m a Lipschitz function V [m ]on T 2.Typical examples for V include nonlocal smoothing operators.The time-dependent analogue of system (1)–(3),also considered in [11,12,13],is∂u ∂t −νΔu +H (x,∇u )=V [m ]in T 2×(0,T ),(4)∂m ∂t +νΔm +div m ∂H ∂p (x,∇u ) =0in T 2×(0,T ),(5)T 2m (x,t )dx =1,m >0,(6)u (t =0)=V 0[m (t =0)],m (t =T )=m 0.(7)∗Received by the editors May 11,2009;accepted for publication (in revised form)June 8,2010;published electronically July 29,2010./journals/sinum/48-3/75847.html †UFR Math´e matiques,Universit´e Paris Diderot,Case 7012,175rue du Chevaleret,75013Paris,France and UMR 7598,Laboratoire Jacques-Louis Lions,F-75005,Paris,France (achdou@math.jussieu.fr).‡Dipartimento di Matematica,Sapienza Universit`a di Roma,Piazzale A.Moro 2,I-00185Roma,Italy (capuzzo@mat.uniroma1.it).1136MEAN FIELD GAMES:NUMERICAL METHODS1137 We refer to the mentioned papers of sry and P.-L.Lions for analytical results concerning system(1)–(3)as well as for its interpretation in stochastic game theory. Let us mention here only that a very important feature of the meanﬁeld model above is that uniqueness and stability may be obtained under reasonable assumptions(see [11,12,13]),in contrast with the Nash system describing the individual behavior of each player,for which uniqueness hardly occurs.The aim of the present work is to propose discrete approximations byﬁnite dif-ference methods of the meanﬁeld model,both in the stationary case(1)–(3)or the evolutive one(4)–(7).The numerical schemes that we use rely basically on monotone approximations of the Hamiltonian and on a suitable weak formulation of the equation for m.These schemes have several important features:•Existence and uniqueness for the discretized problems can be obtained by similar arguments as those used in the continuous case.•They are robust whenν→0(the deterministic limit of the models).•Bounds on the solutions,which are uniform in the grid step,can be proved under reasonable assumptions on the data.Let us mention in this respect that an important research activity is currently going on about approximation procedures for meanﬁeld games.Quite recently,we learned about a diﬀerent numerical approach,based on the reformulation of the model as an optimization problem,which is restricted,however,to the case when V[m](x)= g(m(x));see[10].See also[5]for a recent work on discrete time,ﬁnite state space meanﬁeld games.In section2,we present the approximation of the nonlinear operators involved in,e.g.,(1)–(3)and the main assumptions that are going to be made.Theﬁnite diﬀerence scheme for the stationary model is discussed in section3.Emphasis is put on existence and uniqueness and on bounds on the solution;the main diﬃculty faced there is to obtain bounds on the solution which are uniform in the discretization parameters.An example of a convergence result is also supplied in section3.In section4we discuss an implicit in timeﬁnite diﬀerence method for the following evolution problem comprising two forward parabolic equations:∂u ∂t −νΔu+H(x,∇u)=V[m]in T2×(0,T),(8)∂m ∂t −νΔm−divm∂H∂p(x,∇u)=0in T2×(0,T),(9)T2m(x,t)dx=1,m>0,(10)u(x,0)=u0(x),m(x,0)=m0(x).(11)By analogy with known results long time approximations for the cell problem in homogenization(see[14]),we expect that there exists someλ∈R such that u(x,t)−λt and m(x,t)converge,as t→+∞,to the solution(u(x),m(x),λ)of the stationary system(1)–(3).The main result in section4is Theorem5on the existence for the discrete system.section5deals with a semi-implicit scheme for the evolution system (4)–(7)and contains results on existence and uniqueness.Finally,the long time strategy mentioned above and the numerical experiments for the stationary models are described in section6.A Newton method for the evolutive problem(4)–(7)will be discussed in a forth-coming work(see[1]).1138YVES ACHDOU AND ITALO CAPUZZO-DOLCETTA2.Theﬁnite diﬀerence operators.2.1.The proposed schemes.For simplicity,we discuss the approximation of (1)–(3).Let T2h be a uniform grid on the torus with mesh step h(assuming that1/h is an integer N h)and x ij denote a generic point in T2h.The values of u and m at x i,j will be approximated by U i,j and M i,j,respectively.Hereafter,we will often make the following assumptions on the operator V:(A1):V:m→V[m]maps the set of probability measures into a bounded set of Lipschitz functions on T2.(A2):If m n converges weakly to m,then V[m n]converges to V[m]uniformly on T2.A possible approximation of V[m](x i,j)is(12)(V h[M])i,j=V[m h](x i,j),calling m h the piecewise constant function taking the value M i,j in the square|x−x i,j|∞≤h/2,and assuming that V[m h]can be computed in practice.We introduce theﬁnite diﬀerence operators(13)(D+1U)i,j=U i+1,j−U i,jhand(D+2U)i,j=U i,j+1−U i,jhand the numerical Hamiltonian g:T2×R4→R.Theﬁnite diﬀerence approximation of H(x,∇u)is g(x i,j,(D+1U n+1)i,j,(D+1U n+1)i−1,j,(D+2U n+1)i,j,(D+2U n+1)i,j−1). Classically,we choose the discrete version of(1)as(14)−ν(Δh U)i,j+g(x i,j,[D h U]i,j)+λ=(V h[M])i,jwith the notations(Δh W)i,j=−1h2(4W i,j−W i+1,j−W i−1,j−W i,j+1−W i,j−1),(15)[D h W]i,j=(D+1W)i,j,(D+1W)i−1,j,(D+2W)i,j,(D+2W)i,j−1T.(16)We make the following assumptions on the discrete Hamiltonian g:(q1,q2,q3,q4)→g(x,q1,q2,q3,q4):(H1):Monotonicity:g is nonincreasing w.r.t.q1and q3and nondecreasing w.r.t. q2and q4.(H2):Consistency:(17)g(x,q1,q1,q2,q2)=H(x,q)∀x∈T2∀q=(q1,q2)∈R2.(H3):Diﬀerentiability:g is of class C1.The discrete version of(2)is chosen according to the following heuristics:•When u isﬁxed,(2)is a linear elliptic equation for m.Therefore,when U is ﬁxed,the discrete version of(2)should yield a matrix with positive diagonal entries and nonpositive oﬀ-diagonal entries so that hopefully a discrete maximum principle may be used.•The argument used in[13,11,12]for the uniqueness of(1)–(3)and(4)–(7)should hold in the discrete cases.For this reason,the discrete Hamiltonian g introduced above should be used in the discrete version of(2)as well,and we will often make another assumption on g.(H4):Convexity:the function(q1,q2,q3,q4)→g(x,q1,q2,q3,q4)is convex.MEAN FIELD GAMES:NUMERICAL METHODS 1139The main idea is to consider the weak form of (2).It involves the term − T 2div m ∂H ∂p (x,∇u ) w = T2m ∂H ∂p (x,∇u )·∇w which will be approximated by h 2 i,jm i,j ∇q g (x i,j ,[D h U ]i,j )·[D h W ]i,j .This yields the following discrete version of (9):(18)−ν(Δh M )i,j −1h ⎛⎜⎝M i,j ∂g ∂q 1(x i,j ,[D h U ]i,j )−M i −1,j ∂g ∂q 1(x i −1,j ,[D h U ]i −1,j )+M i +1,j ∂g ∂q 2(x i +1,j ,[D h U ]i +1,j )−M i,j ∂g ∂q 2(x i,j ,[D h U ]i,j )⎞⎟⎠−1h ⎛⎜⎝M i,j ∂g ∂q 3(x i,j ,[D h U ]i,j )−M i,j −1∂g ∂q 3(x i,j −1,[D h U ]i,j −1)+M i,j +1∂g ∂q 4(x i,j +1,[D h U ]i,j +1)−M i,j ∂g ∂q 4(x i,j ,[D h U ]i,j )⎞⎟⎠⎫⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎬⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎭=0.We will also use the more compact but less explicit notation(19)B i,j (U,M )=1h ⎛⎜⎜⎜⎜⎜⎜⎜⎜⎜⎜⎜⎝⎛⎜⎝M i,j ∂g ∂q 1(x i,j ,[D h U ]i,j )−M i −1,j ∂g ∂q 1(x i −1,j ,[D h U ]i −1,j )+M i +1,j ∂g ∂q 2(x i +1,j ,[D h U ]i +1,j )−M i,j ∂g ∂q 2(x i,j ,[D h U ]i,j )⎞⎟⎠+⎛⎜⎝M i,j ∂g ∂q 3(x i,j ,[D h U ]i,j )−M i,j −1∂g ∂q 3(x i,j −1,[D h U ]i,j −1)+M i,j +1∂g ∂q 4(x i,j +1,[D h U ]i,j +1)−M i,j ∂g ∂q 4(x i,j ,[D h U ]i,j )⎞⎟⎠⎞⎟⎟⎟⎟⎟⎟⎟⎟⎟⎟⎟⎠,which makes it possible to write (18)in a shorter way:(20)−ν(Δh M )i,j −B i,j (U,M )=0.Remark 1.It is important to realize that the operator M →−ν(Δh M )i,j −B i,j (U,M )is the adjoint of the linearized version of the operator U →−ν(Δh U )i,j +g (x i,j ,[D h U ]i,j ).(H 5):A further consistency assumption:One has to assume that the discrete operator in (20)is a consistent approximation of the diﬀerential operator in (2),i.e.,that there exists a positive integer ,a real number δ0,δ0∈(0,1),and some positive real number r such that for every v,m ∈C ,δ0(T 2),there is a constant K depending on the norms of v and m in the previously mentioned Schauder spaces such that for all h <1,calling V and M the grid functions deﬁned by V i,j =(1/h 2) |x −x i,j |∞<h/2vdx and M i,j =(1/h 2) |x −x i,j |∞<h/2mdx ,we have for all i,j (21) B i,j ( V , M )−div m ∂H ∂p(x,∇v ) (x i,j ) ≤Kh r .This assumption is clearly fulﬁlled if g satisﬁes (17)and if g and H are smooth enough.1140YVES ACHDOU AND ITALO CAPUZZO-DOLCETTA2.2.Summary.Finally,the ﬁnite diﬀerence approximation of (1)–(3)is to look for two grid functions U,M on T 2h and for a scalar λsuch that−ν(Δh U )i,j +g (x i,j ,[D h U ]i,j )+λ=(V h [M ])i,j∀i,j :0≤i,j <N h ,(22)−ν(Δh M )i,j −B i,j (U,M )=0∀i,j :0≤i,j <N h ,(23)i,j U i,j =0,(24)h 2i,j M i,j =1and M i,j ≥0for 0≤i,j <N h(25)with (V h [M ])i,j deﬁned in (12),B i,j (U,M )deﬁned in (19),and the numerical Hamil-tonian g :T 2×R 4→R satisfying at least (H 1)–(H 3)above.The same strategy will be used to approximate the evolutive problems (4)–(7)and(8)–(11)with an implicit scheme;see,respectively,(75)–(79)and (61)–(62)below.For two grid functions W and Z ,we deﬁne the inner product (W,Z )2= i,j W i,j Z i,j .2.3.A useful lemma.We recall a useful lemma,which can be found in,e.g.,[4].We give its proof for completeness.Lemma 1.Let V be a grid function on T 2h and ρbe a positive parameter.Assume that g satisﬁes (H 1)–(H 3).There exists a unique grid function U such that(26)ρU i,j +g (x i,j ,[D h U ]i,j )−ν(Δh U )i,j =V i,j .Proof .Existence for (26)is proved by using the Leray–Schauder ﬁxed point theorem.Indeed,we consider the mapping F :R N 2h →R N 2h ,(F (U ))i,j =1ρ(ν(Δh U )i,j −g (x i,j ,[D h U ]i,j )+V i,j ),and the real number r =max (i,j )|H (x i,j ,0)−V i,j |/ρ.From the continuity of g ,F is continuous from B r ={U ∈R N 2h : U ∞≤r }to R N 2h .Assuming that U ∈∂B r ,there must exist at least one pair of indices (i 0,j 0)such that U i 0,j 0=±r .Assuming that U i 0,j 0=r ,we haveν(Δh U )i 0,j 0−g (x i 0,j 0,[D h U ]i 0,j 0)≤−H (x i 0,j 0,0)from the monotonicity and the consistency of g .Hence,(F (U ))i 0,j 0≤1ρ(−H (x i 0,j 0,0)+V i 0,j 0)≤r and (F (U ))i 0,j 0=λU i 0,j 0whenever λ>1.Similarly,if U i 0,j 0=−r ,then (F (U ))i 0,j 0≥−r which implies that (F (U ))i 0,j 0=λU i 0,j 0.Therefore,F (U )=λU for all λ>1and U ∈∂B r .The Leray–Schauder ﬁxed point theorem can be used;there exists a solution of (26)in B r .Uniqueness for (26)stems from the monotonicity of g .3.Numerical analysis of the stationary problem (22)–(25).Existence results for (22)–(25)can be proved under additional assumptions on g and V h .The strategy will be to apply the Brouwer theorem to a map χdeﬁned on the compact and convex set (27)K =⎧⎨⎩(M i,j )0≤i,j<N h :h 2 i,jM i,j =1,M i,j ≥0⎫⎬⎭which can be viewed as the set of the discrete probability measures.MEAN FIELD GAMES:NUMERICAL METHODS1141 We will see below that existence can be proved without bounds on U uniform with respect to h since the problem isﬁnite dimensional.However,when possible,we will insist much on obtaining such bounds,for example,equicontinuity with respect to h,because they are important for passing to the limit when h→0.Weﬁrst deﬁne a mapΦ:M∈K→U,where(U,λ)is the unique solution of(22) subject to the constraint in(24).The map M→χ(M)is then obtained by solving a perturbation of(23)with U=Φ(M),subject to the constraints in(25).The discrete function U=Φ(M)will be obtained by passing to the limit in the following Hamilton–Jacobi–Bellman equation(hereafter the HJB equation)(28)ρU(ρ)i,j+g(x i,j,[D h U(ρ)]i,j)−ν(Δh U(ρ))i,j=(V h[M])i,jwhen the positive parameterρtends to0.Such a strategy is reminiscent of those used for solving the cell problems in the homogenization of HJB equations;see,e.g., [14,2,3].Weﬁrst need to study(28)and obtain some bounds on Uρuniform w.r.t.ρand M(and possibly uniform w.r.t.h);these will yield bounds on U uniform w.r.t. M(and possibly uniform w.r.t.h).3.1.Preliminary results.Concerning the continuous problem,one of the as-sumptions made in[13,11]was that there existsθ∈(0,1)such that for|p|large,(29)infx∈T2∇x H·p+θ2νH2>0.It was useful in order to apply Bernstein’s method to(1)and get a bound on ∇u ∞. With assumption(29),we were not able to extend the method of Bernstein to the discrete level.Several other assumptions on H and g can be made.Assumption1 below will make it possible to use the results of Kuo and Trudinger[9]and[8]on H¨o lder estimates for the solution of(28).The slightly stronger Assumption2will make it possible to apply the recent results of Krylov[7].We will use the following notation:(D c1U)i,j=U i+1,j−U i−1,j2h=12(D+1U)i,j+(D+1U)i−1,j,(D c2U)i,j=U i,j+1−U i,j−12h=12(D+2U)i,j+(D+2U)i,j−1,(D21U)i,j=U i+1,j+U i−1,j−2U i,jh2=1h(D+1U)i,j−(D+1U)i−1,j,(D22U)i,j=U i,j+1+U i,j−1−2U i,jh2=1h(D+2U)i,j−(D+2U)i,j−1.Assumption1.(a)The Hamiltonian H is of the form(30)H(x,p)=maxα∈A(p·α−L(x,α)),where A is a compact subset of R2and L is a C0function on T2×A.The function H is continuous with respect to x and of class C1with respect to p.(b)The discrete Hamiltonian g:T2×R4→R,(x,q)→g(x,q),is continuous with respect to x uniformly in h.For all h≤h0,it satisﬁes(H1),(H2),and(H3).1142YVES ACHDOU AND ITALO CAPUZZO-DOLCETTA(c)Deﬁning the function F :T 2×R 4→R ,(x,q 1,q 2,s 1,s 2)→F (x,q 1,q 2,s 1,s 2)by(31)−ν(Δh U )i,j +g (x i,j ,[D h U ]i,j )=F (x i,j ,(D c 1U )i,j ,(D c 2U )i,j ,(D 21U )i,j ,(D 22U )i,j ),we assume that there exist positive constants a 0,a 1,and b 0such that for h =1/N h ≤h 0,(32)a 0≤−∂F ∂s i ≤a 1and ∂F ∂q i≤b 0.(d)There exists a function g ∞:R 4→R such that •g ∞(x,0)=0,•for all q ∈R 4,lim →0sup x ∈T 2| g (x,q )−g ∞(q )|=0,•g ∞is nonincreasing with respect to q 1and q 3and nondecreasing with respect to q 2and q 4.Example 1.Let H be given by (30)with A ={α∈R 2,|α|≤1}and L (x,α)=L (α)=|α|γ/γwith γ>1.It can be seen that H (x,p )=H (p )=⎧⎪⎨⎪⎩γ−1γ|p |γγ−1,|p |≤1,|p |−1γ,|p |≥1and that with the Godunov scheme g (x,q 1,q 2,q 3,q 4)=H (q −1)2+(q −3)2+(q +2)2+(q +4)2 ,Assumption 1holds with g ∞(x,q 1,q 2,q 3,q 4)= (q −1)2+(q −3)2+(q +2)2+(q +4)2in point (d).Assumption 2.(a)The Hamiltonian H is of the form (30),where A is a compact subset of R 2and L is a C 0function on T 2×A .The function H is Lipschitz continuous with respect to x uniformly in p and of class C 1with respect to p .(b)The discrete Hamiltonian g satisﬁes point (b)in Assumption 1.(c)The discrete Hamiltonian g is of the form (33)g (x,q 1,q 2,q 3,q 4)=sup β∈B 4 =1(−a (x,β)s +b (x,β)q )−f (x,β) ,where•B is a compact set,•s 1=s 2=(q 1−q 2)/h ,s 3=s 4=(q 3−q 4)/h ,•a 1=a 2≥0and a 3=a 4≥0,•the functions a ,b :T 2×B →R are continuous w.r.t.β(uniformly w.r.t.h ),and b and ν2+a are Lipschitz continuous w.r.t.x (uniformly w.r.t.h ),•the function f :T 2×B →R is continuous w.r.t.βand Lipschitz continuous w.r.t.x ,•for all h ≤h 0,(x,β)∈T 2×B ,max hb +1(x,β)−a 1(x,β),hb −2(x,β)−a 2(x,β),hb +3(x,β)−a 3(x,β),hb −4(x,β)−a 4(x,β)≤0.(d)The discrete Hamiltonian g satisﬁes point (d)in Assumption 1.MEAN FIELD GAMES:NUMERICAL METHODS 1143Example 2.We take H as in Example 1.The Lax–Friedrichs scheme with a large enough artiﬁcial viscosity parameter θsatisﬁes Assumption 1.It also satisﬁes Assumption 2becauseg (x,q 1,q 2,q 3,q 4)=H x, q 1+q 22,q 3+q 42 −θ(q 1−q 2+q 3−q 4)=sup α∈Aα1q 1+q 22+α2q 3+q 42−θ(q 1−q 2+q 3−q 4)−L (α) .Example 3.We give a simple example where H depends only on p 1.Let H be given by (30)with A ={(α,0),|α|≤1}and L (x,(α,0))=|α|θ/θwith θ>1.It can beseen that H (x,p )=H (p 1)with H (p 1)=θ−1θ|p 1|θθ−1if |p 1|≤1and H (p 1)=|p 1|−1θif |p 1|≥1.Consider the discrete Hamiltonian g (x,q 1,q 2,q 3,q 4)=max |β1|≤1,|β2|≤1−β−1q 1+β+2q 2−1θ|β1|θ−1θ|β2|θ .Assumption 2holds.In particular,g ∞(x,q 1,q 2,q 3,q 4)=max(q 2,−q 1,q 2−q 1,0).Proposition 1.Assume that H and g satisfy Assumptions 1or 2.Let V be a grid function on T 2h (we agree to write V instead of V h )and ρbe a nonnegative real number.For h ≤h 0,let the grid function U on T 2h be the solution of(34)ρU i,j +g (x i,j ,[D h U ]i,j )−ν(Δh U )i,j =V i,j ;see Lemma 1.If Assumption 1holds and if V ∞is bounded uniformly w.r.t.h ≤h 0by a constant c 0,then there exist two constants δ,δ∈(0,1)and C ,C >0,both depending on a 0,a 1,b 0,c 0and on U ∞but not on h and ρsuch that for all h ≤h 0,0<ρ≤1,(35)|U (ξ)−U (ξ )|≤C |ξ−ξ |δ∀ξ,ξ ∈T 2h .If Assumption 2holds and if(36) V ∞+ D h V ∞is bounded uniformly w.r.t.h ≤h 0by a constant c 0,there exists a constant C depending on U ∞but not on h and ρsuch that for all h ≤h 0,0<ρ≤1,(37)|U (ξ)−U (ξ )|≤C |ξ−ξ |∀ξ,ξ ∈T 2h .Proof .In the ﬁrst case,the result is a consequence of a theorem due to Kuo and Trudinger;see formula (3.10)in [9]and also [8](which makes use of (32)).In the second case,(37)is a particular case of a discrete Lipschitz estimate recently proved by Krylov with a very clever discrete Bernstein method (see [7],Theorem 2.5and Remark 4.5).Remark 2.To cast the discrete quasi-linear operator into the setting of Theo-rem 2.5in [7],one needs to consider the grid function W i,j =−U i,j .In Proposition 1the constants C depend on U ρ ∞.It is possible to improve this result by realizing that the constants actually depend on U ρ−U ρ0,0 ∞and by showing that this quantity is bounded uniformly w.r.t.ρand h .The following proposition is due to Camilli and Marchi;see [3].We give its proof for completeness.1144YVES ACHDOU AND ITALO CAPUZZO-DOLCETTAProposition2.1.If Assumption1holds and if V ∞is bounded uniformly w.r.t.h≤h0bya constant c0,then there exist two constantsδ,δ∈(0,1)and C>0,bothindependent of h≤h0andρsuch that for allρ,1≥ρ>0,the solution of(34)satisﬁes(38)maxξ=ξ ∈T2h |U(ρ)(ξ)−U(ρ)(ξ )||ξ−ξ |δ≤C.2.If Assumption2and(36)hold,then there exists a constant C independent ofh≤h0andρsuch that for allρ,1≥ρ>0,the solution of(34)satisﬁes(39)maxξ=ξ ∈T2h |U(ρ)(ξ)−U(ρ)(ξ )||ξ−ξ |≤C.Proof.We give the proof in only theﬁrst case since the second case is done similarly.Lemma1yields existence for(34).We also easily obtain a bound on ρU(ρ) ∞, namely,that(40) ρU(ρ) ∞≤maxi,j(|H(x i,j,0)|+|V i,j|)so there exists a positive constant C1independent of h andρsuch that ρU(ρ) ∞≤C1. Let us haveρtend to0.We set W(ρ)=U(ρ)−U(ρ)0,0.Fixing h,assume that there exists a sequenceρk such that lim k→∞ W(ρk) ∞=+∞.We use the notation k= 1/ W(ρk) ∞.The grid function Z(k)= k W(ρk)satisﬁesZ(k) 0,0=0, Z(k) ∞=1,(41)ρk k Z(k)i,j−νk(Δh Z(k))i,j+gx i,j,1k[D h Z(k)]i,j+ρk U(ρk)0,0=V i,j.(42)But(42)is equivalent to(43)ρk Z(k)i,j −ν(Δh Z(k))i,j+ k gx i,j,1k[D h Z(k)]i,j+ρk k U(ρk)0,0= k V i,j.Note that−ν(Δh Z(k))i,j+ k gx i,j,1k[D h Z(k)]i,j=G(x i,j,(D c1Z)i,j,(D c2Z)i,j,(D21Z)i,j,(D22Z)i,j),where G(x,q1,q2,s1,s2)= F(x,q1/ ,q2/ ,s1/ ,s2/ ),so G also satisﬁes estimate(32).From this and(40),we can apply estimate(35)to Z(k);we get that the grid func-tions Z(k)are equibounded and equicontinuous.Up to a subsequence,Z(k)converges to Z as k tends to inﬁnity and Z satisﬁesZ0,0=0and−ν(Δh Z)i,j+g∞(x i,j,[D h Z]i,j)=0∀i,j.The assumptions made above on g∞and the discrete maximum principle yield that Z=0,which contradicts(41).We have proved that U(ρ)−U(ρ)0,0 ∞≤C for a constant C independent ofρ,and(38)is a consequence of Proposition1.MEAN FIELD GAMES:NUMERICAL METHODS 1145It can be shown by a similar contradiction argument using the Ascoli–Arzel`a theorem that the constant C in the bound U (ρ)−U (ρ)0,0 ∞≤C does not depend on h because the unique viscosity solution of −νΔz +sup α∈A α.∇z =0with z (0)=0is 0.3.2.Existence for the discrete problem.Theorem 1.If Assumption 1is satisﬁed and if the operator V maps the proba-bility measures to a bounded set of continuous functions on T 2and satisﬁes (A 2),then the discrete problem (22)–(25)has at least a solution and there exist two constants δ,δ∈(0,1)and C >0such that (s.t.)for all h =1/N h <h 0,(44) U ∞+max ξ=ξ ∈T 2h|U (ξ)−U (ξ )||ξ−ξ |δ≤C.Proof .Step 1.We consider a mapping Φ:M ∈K →U ,where U is part of the solution of the problem.Find a grid function U and a scalar λsuch that(45)−ν(Δh U )i,j +g (x i,j ,[D h U ]i,j )+λ=(V h [M ])i,jwith i,j U i,j =0.Indeed,it can be proved that if g satisﬁes the assumptions mentioned above,then there exist a unique λ∈R and a unique grid function U satisfying (45).To do it,we pass to the limit in (28)as ρ→0.Existence and uniqueness for (28)stem from Lemma 1.We may apply Proposition 2since V h [M ]is bounded uniformly w.r.t.h and M .Proposition 2implies that there exist two constants C >0and δ∈(0,1)independent of h ,M ,and ρsuch that (38)holds.Thus,there exists a constant c (h )∼h δ−1independent of M and ρsuch that(46) D h U (ρ) ∞≤c (h ).Up to the extraction of a subsequence,we may say that as ρtends to 0,U (ρ)−h 2 i,j U (ρ)i,j converges to a grid function U such that i,j U i,j =0and that ρh 2 i,j U (ρ)i,j converges to λ∈R .It is an easy matter to check that (U,λ)satisﬁes (45)and that the bounds (38)and (46)hold for U .Uniqueness for λstems from the following comparison principle:If U is a sub-solution of (45)with λ=λ1and W is a supersolution of (45)with λ=λ2,then λ2≤λ1.Uniqueness for U is obtained by repeatedly applying the discrete maximum principle from the monotonicity of g .We have deﬁned the map Φ:M ∈K →U ,where (U,λ)solve (45)and i,j U i,j =0.Step 2:continuity of Φ.Consider a sequence of grid functions M (k )in K which tends to M ∈K as k tends to inﬁnity.From the assumptions on V and V h ,V h [M (k )]converges to V h [M ].Consider λand U a solution of (45),and call λ(k ),U (k )a solution of (45)with M =M (k ).From the estimates above,the sequences (λk )k and ( U (k ) ∞)k are bounded.One can extract a subsequence k such that λ(k )tends to λand U (k )tends to U and such that −ν(Δh U )i,j +g (x i,j ,[D h U ]i,j )+ λ=(V h [M ])i,j∀i,j,and i,j U i,j =0.Uniqueness for (45)implies that λ=λand U =U .The whole sequences (λ(k ))k ,(U (k ))k therefore tend to λ,U .We have proved that the map Φis continuous.Step 3.For M ∈K and U =Φ(M ),consider the following linear problem.Find M such that (47)μ Mi,j −ν(Δh M )i,j −B i,j (U, M )=μM i,j ,where μis a suﬃciently large positive number which will be chosen later.This linear problem may be written (48)μ M+A M =μM,where A is a linear operator depending on U .The assumptions of the monotonicity of g imply that∂g∂q 1≤0,∂g∂q 2≥0,∂g ∂q 3≤0,and ∂g∂q 4≥0.This yields that the matrix corresponding to A has positive diagonal entries and nonpositive oﬀ-diagonal entries.Furthermore,since g is C 1,(46)implies that there exists a constant C independent of M (but possibly on h )such that for all i,j ,0≤i,j ≤N h ,and for all =1,2,3,4,(49)∂g∂q(x i,j ,[D h U ]i,j ) ≤C.From this,we see that for μlarge enough depending possibly on h but not on M ,the matrix corresponding to μId +A is an M-matrix and is therefore invertible.Thesystem of linear equations (47)has a unique solution M,and M is nonnegative since M is nonnegative.We are left with proving that h 2( M,1)2=h 2(M,1)2=1.For two grid functions W and Z ,let us compute (AW,Z )2.Discrete integrations by part lead to(AW,Z )2=ν i,j(D +1W )i,j (D +1Z )i,j +(D +2W )i,j (D +2Z )i,j +i,jW i,j [D h Z ]i,j ·∇q g x i,j ,[D h U ]i,j.(50)It is easy to check that for all grid functions W ,(AW,1)2=0.Therefore,takingthe inner product of (48)with the function Z =1,we obtain that h 2( M,1)2=h 2(M,1)2=1,so M∈K .We call χ:K →K the mapping deﬁned by χ:M → M.Step 4:existence of a ﬁxed point of χ.From the boundedness and continuity of the mapping Φ,and from the fact that g is C 1in the variable q ,we obtain that χis continuous.Therefore,we can apply Brouwer’s ﬁxed point theorem and obtain that χhas a ﬁxed point.We obtain a better result under Assumption 2and stronger assumptions on V .Theorem 2.If Assumption 2holds and if V satisﬁes (A 1)and (A 2),then the discrete problem (22)–(25)has at least a solution and there exists a constant C such that for all h =1/N h <h 0,(51)U ∞+max ξ=ξ ∈T 2h|U (ξ)−U (ξ )||ξ−ξ |≤C.Proof .The proof is similar to that of Theorem 1,using now the second part ofProposition 2.Since the discrete problem is ﬁnite dimensional,existence for (22)–(25)can be proved without a bound on U uniform with respect to h .Diﬀerent assumptions on the structure of g can be made;for example,see the following theorem.Theorem 3.Assume that•g satisﬁes (H 1),(H 2)and (H 3),•there exist two positive constants α>0and γ>1and a nonnegative constant C such that (52)g (x,q 1,q 2,q 3,q 4)≥α((q −1)2+(q +2)2+(q −3)2+(q +4)2)γ/2−C ∀x ∈T 2,•V maps the probability measures to a bounded set of continuous functions onT 2and satisﬁes (A 2).The discrete problem (22)–(25)has at least a solution.Proof .The proof follows the same steps as that of Theorem 1.Only the ﬁrst step of the proof is modiﬁed as follows.Existence and uniqueness for (28)follow from Lemma 1.We also easily obtain a bound on ρU (ρ) ∞,namely,that(53) ρU (ρ)∞≤max i,jH (x i,j ,0)+(V h [M ])i,j ,so there exists a positive constant C 1independent of M and ρsuch that ρU (ρ) ∞≤C 1.From this,we deduce that there exists a positive constant C 2independent of M and ρsuch that (54)g (x i,j ,[D h U (ρ)]i,j )−ν(Δh U (ρ))i,j ≤C 2∀i,j.Using (52),we see thatg (x i,j ,[D h W ]i,j )−ν(Δh W )i,j≥α ((D +1W )−i,j )2+((D +1W )+i −1,j )2+((D +2W )−i,j )2+((D +2W )+i,j −1)2 γ/2−C −νh(D +1W )+i,j +(D +1W )−i −1,j +(D +2W )+i,j +(D +2W )−i,j −1 .Calling P ρ= D h U (ρ) ∞=max i,j max(|(D +1U (ρ))i,j |,|(D +2U (ρ))i,j |),we deduce from (54)and the previous estimate that there exists a constant C 3independent of M and ρsuch thatαP γρ−4νhP ρ≤C 3.This yields (46)for a constant c (h )independent of M and ρ.Up to the extractionof a subsequence,we may say that as ρtends to 0,U (ρ)−h 2 i,j U (ρ)i,j converges toa grid function U such that i,j U i,j =0and that ρh 2 i,j U (ρ)i,j converges to λ∈R .The limits U and λsatisfy (45).Uniqueness for (45)is proved as above,so Φis well deﬁned.Remark 3.In Theorem 3,we were not able to obtain an estimate on U uniform w.r.t.h .Remark 4.Note that the assumption (A 2)can be relaxed in the discrete case.Indeed,Theorem 1holds if we replace (A 2)with the assumption that V h is a continu-ous map from K deﬁned in (27)to grid functions bounded by a constant independent of h .Theorem 3holds if V h is a continuous map from K to grid functions.These observations lead to existence results when V h is a local operator;see section 3.4.。

高二英语概率计算单选题30题及答案

高二英语概率计算单选题30题及答案1.The probability of winning a certain lottery is 1/10. What is the probability of not winning?A.1/10B.9/10C.1/9D.8/9答案：B。

解析：概率总和为1，不中奖的概率等于1 减去中奖概率，1 减去1/10 等于9/10。

A 选项是中奖概率；C 选项和D 选项计算错误。

2.A die is rolled. What is the probability of getting an even number?A.1/2B.1/3C.2/3D.1/4答案：A。

解析：骰子有六个面，分别是1、2、3、4、5、6，其中偶数有2、4、6 三个，所以概率是3/6 即1/2。

B 选项是得到1 的概率；C 选项和D 选项计算错误。

3.There are 5 red balls and 3 blue balls in a box. What is the probability of drawing a red ball?A.5/8B.3/8C.1/2D.2/3答案：A。

解析：总共有8 个球，其中红球有5 个，所以概率是5/8。

B 选项是蓝球的概率；C 选项和D 选项计算错误。

4.A card is randomly drawn from a deck of 52 cards. What is the probability of getting a heart?A.1/4B.1/52C.1/13D.1/2答案：A。

解析：一副牌有52 张，其中红心有13 张，所以概率是13/52 即1/4。

B 选项是一张特定牌的概率；C 选项是抽到某一个点数的概率；D 选项计算错误。

5.Two coins are tossed. What is the probability of getting two heads?A.1/4B.1/2C.3/4D.1答案：A。

(完整word版)概率论与数理统计(英文)

3. Ran dom Variables3.1 Defin iti on of Ran dom VariablesIn engin eeri ng or scie ntific problems, we are not only in terested in the probability of events, but also interested in some variables depending on sample points.（定义在样本点上的变量）For example, we maybe in terested in the life of bulbs produced by a certain company, or the weight of cows in a certain farm, etc. These ideas lead to the definition of random variables.1. ran dom variable defi niti onExample 3.1.1 A fair die is tossed. The number X shown is a random variable, it takes values in the set{1,2,L 6}. WExample 3.1.2 The life t of a bulb selected at random from bulbs produced by company A is a random variable, it takes values in the interval（o , ）.W Since the outcomes of a ran dom experime nt can not be predicted in adva nee, the exact value of a ran dom variable can not be predicted beforethe experiment, we can only discuss the probability that it takes somevalue or the values in some subset of R.2. Distribution functionDefinition 3.1.2 Let X be a random variable on the sample space S. Then the fun cti onF(X) P(X x). x Ris called the distribution function of XNote The distribution function F(x)is defined on real numbers, not on sample space.Example 3.1.3 Let X be the number we get from tossing a fair die. Then the distribution function of X is (Figure 3.1.1)0, if x 1;nF (x) , if n x n 1,n 1,2,L ,5;61, if x 6.P11门3. Properties 1 2 3 4 5b "Figure 3.1.1 The distributio n fun cti on in ExampleThe distribution function F(x)of a random variable X has the followi ng properties:(1) F(x) is non-decreasing.In fact, if x 1 x 2, the n the eve nt {Xis a subset ofthe eve nt{X x 2} ,thusF(xJ P(XX 1)P(XX 2) F(X 2)⑵F()lim F(x)xo,F()Jim F(x)1.(3)For any x 0 R ,li mX x oF(x) F(x o0) F(x )) .This is to say, thedistribution function F (x )of a random variable X is right continuous. Example 3.1.4 Let X be the life of automotive parts produced by company A , assume the distribution function of X is (in hours)xF (x) P(X x) I II e 2000, x 0;0, x 0.Find P(X 2ooo ),p (1ooo X 3000).Question : What are the probabilities P(X 2000) and P(X 2000)Soluti onSoluti on By defi niti on,IIP(X 2000)F(2000)1 e 0.6321 .P(1000 X 3000) P(X 3000) P(X1000)F(1000)(1 e 1.5) (1 e 0.5)Let X1be the total number shown, then the events{ X1 k} contains k 1 sample points, k 234,5 . Thusk 1P(X1 k) , k 2,3,4,536And5{X 1} U{X1 k}k 2so5 5P(X 1) P(X k)k 218P(X 1) 1 P(X 1) 1 3 1Thus0, x 1;5F(x) P(X x) , 1 x 1;181, x 1.1. - •--------- oFigure 3.1.2 The distribution function in Example 3.1.5In this book, we study two kinds of ran dom variables.X X 佝忌丄a n 丄}. LetP(X a n ) P n ,n 1,2,L .(3.2.1)Then we have p n o , n 1,2,L , p n 1.nthe probability distributi on of the discrete ran dom variable X (概率分布)注意随机变量X 的分布所满足的条件(1) P i > 0(2) P 1+P 2+…+P n =1离散型分布函数And the distribution function of X is given byF(x) P(X x) P n(3.2.2)an XFor an experime nt in which a coi n is tossed three times (or 3 coins are tossed on ce), con struct the distributi on ofX. (Let X denote the nu mber of head occurre nee)Soluti onn=3, p=1/2__pr0 1/81 3/82 3/83 1/8Example 2在一次试验中，事件A发生的概率为p,不发生的概率为1-p,用X=0表示事件A没有发生，X=1表示事件A发生，求X的分布。

10-11上概率双语A试卷

1,考试作弊将带来严重后果！华南理工大学期末考试《概率统计（双语）》试卷A2010－2011学年度上学期1. 考前请将密封线内各项信息填写清楚；所有答案请答在试卷,答题卷上。

．考试形式：闭卷；满分：100分．FILLING THE BLANKS (25 points)A fair coin was flipped 3 times and landed tails 3 times, the probability of___________.(2) If the probability density of a random variable X is given by2, 01()0, elsewherekx xf x⎧<<=⎨⎩, k=___________, the probability for X to take a value between 0.5 and 1.5 is___________ .(,)X Yρ=___________ .X has mean μand standard deviation σ,Chebyshev ’s theorem tell us that ()P x k μσ-≥ ___________ .(5) Suppose Y=3X+1,2(,)XN μσ, Thus Y___________.(6) If P(A) = 2/3, P(B) = 1/2, and P(B|A) = 1/3, P(A|B)= ___________ ..(7) LetX be a variable following an exponential distribution with meanλ, the density function of X is ___________.2. COMPUTATIONS: (60 points)(1). A box contains three blue cards and four white cards. Two cards are drawnone at a time.(a) Find the probability that both cards are blue if the draws are made with replacement.(b) Find the probability that both cards are blue if draws are made without replacement.(2). A supermarket is doing an ad compaign by organizing a lucky raffle draw forevery 100 purchasers. The rules is that every one write his own name on a card, put the card into a lottery box, thus there are 100 names in the box. Now every one draw a card from the box without replacement , one by one. The one who draw the card with his own name get a 50% discount on the purchasing. What is the probability that no one will get the discount? (Assume that the 100 purchasers have different names.)(3). Sara has a collection of 7 books: War and Peace, Steppenwolf, One Hundred Years of Solitude, Huckleberry Finn, Lord of the Flies, The Old Man and the Sea, The Grapes of Wrath. If she randomly selects three of her books to read in sequence this month, what is the probability that she will read War and Peace, Steppenwolf, and One Hundred Years of Solitude in that order?(4). A prisoner is trapped in a cell containing 4 doors. For i =1; 2; 3, the ith door leads to a tunnel that returns him to his cell after i days travel. The fourth door leads to freedom after 2 days of travel. Assumedthat he will always select doors 1; 2; 3 and 4 with respective probabilities0.3, 0.3, 0.2, 0.2. What is the expected number of days until the prison reaches freedom.(6). I toss 3 fair coins, and then re-toss all the ones that come up tails. Let X denote the number of coins that come up heads on the first toss, and let Y denote the number of re-tossed coins that come up heads on the second toss.(a) Determine the joint distribution of X and Y , and use it to calculate E(X + Y ).(b) Derive a formula for E(Y|X).(7) . (a)State the central limit theorem.(b) One has 100 light bulbs whose lifetimes are independent exponentialswith mean 3 hours. If the bulbs are used one at a time, with a failed bulbbeing replaced immediately by a new one,approximate the probability that there is still a working bulb after 310 hours.3. PROOF (15 points)(1). Let1,,n X X be a random sample of size n from a population withnonzero mean μ. Show that 11n n a X a X ++ is an unbiased estimator ofμ if and only if 11n a a ++=. And prove that among allestimators of this form,111n X X n n++ has the smallest variance. (2). Let randon variable (0,1)XN be the standard normaldistribution. To prove that22(1)X χ, i.e. 2X follows the chi-squaredistribution with degree of freedom 1v =.(3). Letn X be a sequance of binomial random variables with probability distribution (;,)n b k n p . If for some constant λ, we have lim n n np λ→∞=,toprovelim (;,)(;)n n b k n p p k λ→∞=. (Hint,()(;)!kP X k p k ek λλλ-===is the probility of Poisson distribution)(4). Let continuous variablesX and Y has joint density function (,)f x y .To prove thatX and Y are independent if and only if there are functions(),()g x h y such that (,)()()f x y g x h y =.Standard Normal Distribution注：最后一行分别对应3.1~3.9。

(完整版)概率论与数理统计英文版第三章

Chapter 3. Random Variables and Probability Distribution1.Concept of a Random VariableExample: three electronic components are testedsample space (N: non defective, D: defective)S =｛NNN, NND, NDN, DNN, NDD, DND, DDN, DDD｝allocate a numerical description of each outcomeconcerned with the number of defectiveseach point in the sample space will be assigned a numerical value of 0, 1, 2, or 3.random variable X: the number of defective items, a random quantityrandom variableDefinition 3.1A random variable is a function that associates a real number with each element in the sample space.X: a random variablex : one of its valuesEach possible value of X represents an event that is a subset of the sample spaceelectronic component test:E =｛DDN, DND, NDD｝=｛X = 2｝.Example 3.1Two balls are drawn in succession without replacement from an urn containing 4 red balls and 3 black balls. Y is the number of red balls. The possible outcomes and the values y of the random variable Y ?Example 3.2 A stockroom clerk returns three safety helmets at random to three steel mill employees who had previously checked them. If Smith, Jones, and Brown, in that order, receive one of the three hats, list the sample points for the possible orders of returning the helmets,and find the value m of the random variable M that represents the number of correct matches.The sample space contains a finite number of elements in Example 3.1 and 3.2.another example: a die is thrown until a 5 occurs,F: the occurrence of a 5N: the nonoccurrence of a 5obtain a sample space with an unending sequence of elementsS =｛F, NF, NNF, NNNF, . . .｝the number of elements can be equated to the number of whole numbers; can be countedDefinition 3.2 If a sample space contains a finite number of possibilities or an unending sequence with as many elements as there are whole numbers, it is called a discrete sample space.The outcomes of some statistical experiments may be neither finite nor countable.example: measure the distances that a certain make of automobile will travel over a prescribed test course on 5 liters of gasolinedistance: a variable measured to any degree of accuracywe have infinite number of possible distances in the sample space, cannot be equated to the number of whole numbers.Definition 3.3If a sample space contains an infinite number of possibilities equal to the number of points on a line segment, it is called a continuous sample spaceA random variable is called a discrete random variable if its set of possible outcomes is countable.Y in Example 3.1 and M in Example 3.2 are discrete random variables.When a random variable can take on values on a continuous scale, it is called a continuous random variable.The measured distance that a certain make of automobile will travel over a test course on 5 liters of gasoline is a continuous random variable.continuous random variables represent measured data:all possible heights, weights, temperatures, distance, or life periods.discrete random variables represent count data: the number of defectives in a sample of k items, or the number of highway fatalities per year in a given state.2. Discrete Probability DistributionA discrete random variable assumes each of its values with a certain probabilityassume equal weights for the elements in Example 3.2, what's the probability that no employee gets back his right helmet.The probability that M assumed the value zero is 1/3.The possible values m of M and their probabilities are0 1 31/3 1/2 1/6Probability Mass FunctionIt is convenient to represent all the probabilities of a random variable X by a formula.write p(x) = P (X = x)The set of ordered pairs (x, p(x)) is called the probability function or probability distribution of the discrete random variable X.Definition 3.4The set of ordered pairs (x, p(x)) is a probability function, probability mass function, or probability distribution of the discrete random variable X if, for each possible outcome xExample 3.3 A shipment of 8 similar microcomputers to a retail outlet contains 3 that are defective. If a school makes a random purchase of 2 of these computers, find the probability distribution for the number of defectives.SolutionX: the possible numbers of defective computersx can be any of the numbers 0, 1, and 2.Cumulative FunctionThere are many problem where we may wish to compute the probability that the observed value of a random variable X will be less than or equal to some real number x.Definition 3.5The cumulative distribution F (x) of a discrete random variable X with probability distribution p(x) isFor the random variable M, the number of correct matches in Example 3.2, we haveThe cumulative distribution of M isRemark. the cumulative distribution is defined not only for the values assumed by given random variable but for all real numbers.Example 3.5 The probability distribution of X isFind the cumulative distribution of the random variable X.Certain probability distribution are applicable to more than one physical situation.The probability distribution of Example 3.5 can apply to different experimental situations.Example 1: the distribution of Y , the number of heads when a coin is tossed 4 timesExample 2: the distribution of W , the number of read cards that occur when 4 cards are drawn at random from a deck in succession with each card replaced and the deck shuffled before the next drawing.graphsIt is helpful to look at a probability distribution in graphic form.bar chart;histogram;cumulative distribution.3.Continuous Probability DistributionContinuous Probability distributionA continuous random variable has a probability of zero of assuming exactly any of its values. Consequently, its probability distribution cannot be given in tabular form.Example: the heights of all people over 21 years of age (random variable)Between 163.5 and 164.5 centimeters, or even 163.99 and 164.01 centimeters, there are an infinite number of heights, one of which is 164 centimeters.The probability of selecting a person at random who is exactly 164 centimeters tall and not one of the infinitely large set of heights so close to 164 centimeters is remote.We assign a probability of zero to a point, but this is not the case for an interval. We will deal with an interval rather than a point value, such as P (a < X < b), P (W≥c).P (a≤X≤b) = P (a < X≤b) = P (a≤X < b) = P (a < X < b)where X is continuous. It does not matter whether we include an endpoint of the interval or not. This is not true when X is discrete.Although the probability distribution of a continuous random variable cannot be presented in tabular form, it can be stated as a formula.refer to histogramDefinition 3.6 The function f(x) is a probability density function for the continuous random variable X, defined over the set of real numbers R, ifExample 3.6 Suppose that the error in the reaction temperature, in oC, for a controlled laboratory experiment is a continuous random variable X having the probability density function(a) Verify condition 2 of Definition 3.6.(b) Find P (0 < X≤1).Solution: . . . . . . P (0 < X≤1) = 1/9.Definition 3.7The cumulative distribution F (x) of a continuous random variable X with density function f(x) isimmediate consequence:Example 3.7 For the density function of Example 3.6 findF (x), and use it to evaluate P (0 < x≤1).4. Joint Probability Distributionsthe preceding sections: one-dimensional sample spaces and a single random variablesituations: desirable to record the simultaneous outcomes of several random variables.Joint Probability DistributionExamples1. we might measure the amount of precipitate P and volume V of gas released from a controlled chemical experiment; we get a two-dimensional sample space consisting of the outcomes (p, v).2. In a study to determine the likelihood of success in college, based on high school data, one might use a three-dimensional sample space and record for each individual his or her aptitude test score, high school rank in class, and grade-point average at the end of the freshman year in college.X and Y are two discrete random variables, the joint probability distribution of X and Y isp (x, y) = P (X = x, Y = y)the values p(x, y) give the probability that outcomes x and y occur at the same time.Definition 3.8 The function p(x, y) is a joint probability distribution or probability mass function of the discrete random variables X and Y ifExample 3.8Two refills for a ballpoint pen are selected at random from a box that contains 3 blue refills,2 red refills, and 3 green refills. If X is the number of blue refills and Y is the number of red refills selected, find(a) the joint probability function p(x, y)(b) P [(X, Y )∈A] where A is the region｛(x, y)|x + y≤1｝Solutionthe possible pairs of values (x, y) are (0, 0), (0, 1), (1, 0), (1, 1), (0, 2), and (2, 0).p (x, y) represents the probability that x blue and y red refills are selected.present the results in Table 3.1Definition 3.9The function f(x, y) is a joint density function of the continuous random variables X and Y ifWhen X and Y are continuous random variables, the joint density function f(x, y) is a surface lying above the xy plane.P [(X, Y )∈A], where A is any region in the xy plane, is equal to the volume of the right cylinder bounded by the base A and the surface.Example 3.9 Suppose that the joint density function is(b) P [(X, Y )∈A]= 13/160marginal distributionp (x, y): the joint probability distribution of the discrete random variables X and Ythe probability distribution p X(x) of X alone is obtained by summing p(x, y) over the values of Y .Similarly, the probability distribution p Y (y) of Y alone is obtained by summing p(x, y) over the values of X.pX (x) and p Y (y): marginal distributions of X and YWhen X and Y are continuous random variables, summations are replaced by integrals.Definition 3.10The marginal distribution of X alone and of Y alone areExample 3.10 Show that the column and row totals of Table3.1 give the marginal distribution of X alone and of Y alone.Example 3.11 Find marginal probability density functionsf X (x) and fy(y)for the joint density function of Example 3.9.The marginal distribution pX (x) [or fX(x)] and px(y) [or fy(y)] are indeed the probability distribution of the individual variableX and Y , respectively.How to verify?The conditions of Definition 3.4 [or Definition 3.6] are satisfied.Conditional distributionrecall the definition of conditional probability:X and Y are discrete random variables, we haveThe value x of the random variable represent an event that is a subset of the sample space.Definition 3.11Let X and Y be two discrete random variables. The conditional probability mass function of the random variable Y , given that X = x, isSimilarly, the conditional probability mass function of the random variable X, given that Y = y, isDefinition 3.11'Let X and Y be two continuous random variables. The conditional probability density function of the random variable Y , given that X = x, isSimilarly, the conditional probability density function of the random variable X, given that Y = y, isRemark:The function f(x, y)/fX (x) is strictly a function of y with x fixed, the function f(x, y)/fy(y) is strictly a function of x with yfixed, both satisfy all the conditions of a probability distribution.How to find the probability that the random variable X falls between a and b when it is known that Y = yExample 3.12 Referring to Example 3.8, find the conditional distribution of X, given that Y = 1, and use it to determineP (X = 0|Y = 1).Example 3.13The joint density for the random variables (X, Y ) where X is the unit temperature change and Y is the proportion of spectrum shift that a certain atomic particle produces is(a)Find the marginal densities fX (x), fy(y), and the conditional density f Y |X (y|x)(b)Find the probability that the spectrum shifts more than half of the total observations, given the temperature is increased to0 .25 unit.(a)(b)Example 3.14 Given the joint density function(a)(b)statistical independenceevents A and B are independent, ifP (B∩A) = P (A)P (B).discrete random variables X and Y are independent, ifP (X = x, Y = y) = P (X = x)P (Y = y)for all (x, y) within their range.The value x of the random variable represent an event that is a subset of the sample space.Definition 3.12 Let X and Y be two discrete random variables, with joint probability distribution p(x, y) and marginaldistributions pX (x)and pY(y), respectively. The random variables X and Y are said to be statistically independent if and onlyifp (x,y) = pX (x)pY(y) for all (x, y) within their range.Definition 3.12' Let X and Y be two continuous random variables, with joint probability distribution f(x, y) and marginaldistributions fX (x) and fY(y), respectively. The random variables X and Y are said to be statisticallyindependent if and only iff (x, y) =fX (x)fY(y) for all (x, y) within their range.The continuous random variables of Example 3.14 are statistically independent. However, that is not the case for the Example 3.13.For discrete variables, requires more thorough investigation. If you find any point (x, y) for which p(x, y) is defined such that≠pX (x)pY(y), the discrete variables X and Y are not statistically independent.p(x, y)Example 3.15Show that the random variables of Example 3.8 are notstatistically independent.the case of n random variablesjoint marginal distributions of two r.v. X1 and X2Definition 3.13Let x1, x2,…, x n be n discrete random variables, with joint probability distribution p(x1, x2,… , x n)and marginal distributions p X1 (x1), p X2 (x2),…, p Xn (x n),respectively.The random variables x1, x2,…, x n are mutually statistically independent,thenfor all (x1, x2,… , x n) within their range.Definition 3.13' Let x 1, x2,…, x n be n continuous randomvariables, with joint probability distribution f(x1, x2,… , x n)and marginal distributions f X1 (x1),f X2 (x2),…, f Xn (x n)respectively. The random variables x1, x2,…, x n are mutually statistically independent, thenfor all(x1, x2,… , x n)within their range.Example 3.16 Suppose that the shelf life , in years, of a certain perishable food product packaged in cardboard containers is a random variable whose probability density function is given by。

ch2_8 概率论英文版

Recall Input
data and find the solutions.
4
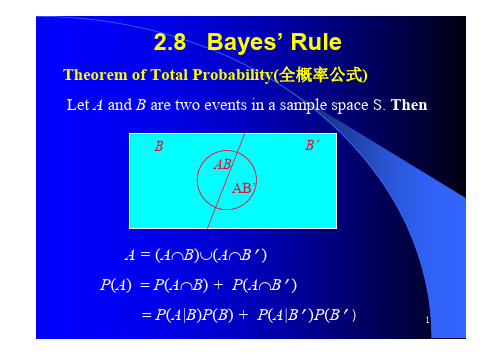
Example 2.38, page 59 In the certain assembly plant, three machines, B1, B2, and B3, make 30%, 45%, and 25%, respectively, of the products. It is known from past experience that 2%, 3%, and 2% of the products made by each machine, respectively, are defective. Suppose that a finished product is randomly selected. What is the probability that it is defective? Solution: D={the product is defective} Bi={the product is made by machine Bi} P(B1)= 30% , P(B2)= 45% , P(B3)= 25% P(D) = P(DB1) + P(DB2) + P(DB3) = P(D|B1)P(B1) + P(D|B2)P(B2) +P(D|B3)P(B3) 5 = 0.02(0.3) + 0.03(0.45) + 0.02(0.25)
k k
P ( A)

i 1
P( A Bi )

i 1
P ( A | Bi ) P ( Bi )
2
Proof:
AB1
AB2 ABi... ABk

ch2_4 概率论英文版

1
Three ways are widely used to assign probabilities:
• Relative frequency approach • Classical approach • Personal approach
2
1. The relative frequency approach
[(5!/3!2!ion
In general: Suppose a population contains n1 individuals of type 1, n2 individuals of type 2, …, nk individuals of type k. If a random sample of size r is taken from the population, then the probability that this sample will contain r1 type 1 individuals, r2 type 2 , …, rk type k individuals is
9
Advantage!
When the outcomes are truly equally likely, the probability is not an approximation, it is an accurate description of frequency with which the event A will occur.
n1 n2 nk ... r r r 1 2 k n r
13
Axioms of Probability (Definition 2.8) 定义
概率的公理化

10-11上概率双语B试卷

1,考试作弊将带来严重后果！华南理工大学期末考试《概率统计（双语）》试卷B 2009－2010学年度下学期1. 考前请将密封线内各项信息填写清楚；所有答案请答在试卷，答题卷上。

．考试形式：闭卷；满分：100分．FILLING THE BLANKS (25 points)(1) A continuous random variable X has distribution function3,()2 0, 0.X a x c F x x c x b x >⎧⎪=≥>⎨⎪≥⎩则a=_________, b=____________, c=____________. The density function()X f x =____________.The expectation of X is ________________. The variance of X is ____________________.3, 01()0, elsewherekx x f x ⎧<<=⎨⎩, k =__________, the probability for X to take a value between 0.5 and 1.5 is __________.(3) Suppose D(X)=36, D(X-Y)=62, D(X+Y)=42. (,)X Y ρ=__________.(4) If a random variableX has mean μ and standard deviation σ,Chebyshev ’s theorem tell us that ()P x με-≤__________.(6) Suppose the pair{},X Y is independent, ,X Y have the uniformdistribution in the interval(0,2).2(01,0)P X Y X ≤≤≤≤=__________.(7) LetX be a variable following an exponential distribution with variance2λ,the density function ofX is _________.2. COMPUTATIONS: (60 points)(1). Suppose the number of children born in Ruritania each day is a binomial randomvariable with mean 1000 and variance 100. Assume that the number of children born on any particular day is independent of the numbers of children born on all other days. Whatis the probability that on at least one day this year, fewer than 975 children will be born in Ruritania?Answer: We approximate the number of children born each day by a normal random variable. Letting X denote the number of children born on some speci_ed day, andZ denote a standard normal, we have P(X _ 975) = P(X _ 974:5) = P(X100010_974:5100010 = 2:55) = P(Z _ +2:55) _ :9946. Since each such random variable X (one foreach day) is assumed independent of the others, the probability that 975 or more childrenwill be born on every day of this year is :9946365 _ :1386, and the probability that, on atleast one day this year, fewer than 975 children will be born is close to 1 :1386 _ 86%.(2). Suppose X is uniform on the interval from 1 to 2. Compute the density function and expected value of the random variable Y = 1=X.(3). A factory procuces machines. The cost of each machine produced is $ 1000, and the selling price is $ 3000. If the number of X of machines sold has the distributions as followsHow many machines that the factory should produced to maximize the expectation of its profits.(5).A coin is tossed 50 times. Use the Central Limit Theorem (applied to a binomial random variable) to estimate the probability that fewer than 20 of those tosses comeup heads.3. PROOFS (15 points)(1).(2).(3).f x y.(4). Let continuous variables X and Y has joint density function (,) To prove that X and Y are independent if and only if there are functions.f x yg xh y(),()g x h y such that (,)()()Standard Normal Distribution注：最后一行分别对应3.1~3.9。

高二英语概率计算练习题30题

高二英语概率计算单选题30题1.What is the probability of getting an even number when rolling a fair six-sided die?A.1/2B.1/3C.1/4D.1/6答案：A。

解析：一个标准的六面骰子，偶数有2、4、6 三个，总共有六个面，所以概率为3/6 即1/2。

2.In a lottery with 10 tickets, one is the winning ticket. What is the probability of randomly picking the winning ticket?A.1/10B.1/9C.1/8D.1/7答案：A。

解析：总共有10 张票，其中一张是中奖票，所以概率为1/10。

3.A bag contains 3 red balls and 2 blue balls. What is the probability of drawing a red ball?A.3/5B.2/5C.1/2答案：A。

解析：袋子里一共有5 个球，其中3 个是红球，所以概率为3/5。

4.If you flip a fair coin twice, what is the probability of getting two heads?A.1/2B.1/3C.1/4D.1/6答案：C。

解析：抛一次硬币得到正面的概率是1/2，抛两次都得到正面的概率是1/2 乘以1/2 等于1/4。

5.A box contains 4 apples and 6 oranges. What is the probability of randomly picking an apple?A.2/5B.3/5C.4/10D.2/3答案：C。

解析：总共有10 个水果，其中4 个是苹果，所以概率为4/10。

6.In a deck of 52 playing cards, what is the probability of drawing a heart?A.1/4C.13/52D.4/52答案：A。

高二英语概率计算单选题30题

高二英语概率计算单选题30题1. A box contains 5 red balls and 3 blue balls. What is the probability of drawing a red ball?Answer: The total number of balls is 5 (red) + 3 (blue) = 8. The probability of drawing a red ball is 5/8.2. There are 10 cards numbered from 1 to 10. What is the probability of picking a card with an even number?Answer: There are 5 even numbers (2, 4, 6, 8, 10) out of 10 cards. So the probability is 5/10 = 1/2.3. A bag has 4 green marbles and 6 yellow marbles. What is the probability of choosing a green marble?Answer: The total number of marbles is 4 (green) + 6 (yellow) = 10. The probability of choosing a green marble is 4/10 = 2/5.4. In a deck of cards, what is the probability of drawing a spade?Answer: There are 13 spades in a deck of 52 cards. So the probability is 13/52 = 1/4.5. A jar contains 8 red candies and 4 blue candies. What is the probability of picking a blue candy?Answer: The total number of candies is 8 (red) + 4 (blue) = 12. The probability of picking a blue candy is 4/12 = 1/3.6. A box contains 5 red balls and 3 blue balls. If you randomly pick a ball from the box, what is the probability of getting a red ball? Answer: Thetotal number of balls is 5 (red) + 3 (blue) = 8. The probability of getting a red ball is 5/8.7. There are 10 cards numbered from 1 to 10. If you randomly draw one card, what is the probability that the number on the card is a prime number? Answer: Prime numbers from 1 to 10 are 2, 3, 5, 7. So there are 4 prime numbers. The total number of cards is 10. The probability is 4/10 = 2/5.8. In a bag of marbles, there are 4 green marbles, 6 yellow marbles, and 2 blue marbles. What is the probability of picking a yellow marble? Answer: The total number of marbles is 4 (green) + 6 (yellow) + 2 (blue) = 12. The probability of picking a yellow marble is 6/12 = 1/2.9. A dice is rolled. What is the probability of getting an even number? Answer: There are three even numbers (2, 4, 6) on a dice. The total number of possible outcomes is 6. So the probability is 3/6 = 1/2.10. There are 8 boys and 12 girls in a class. If a student is randomly selected, what is the probability of choosing a girl? Answer: The total number of students is 8 (boys) + 12 (girls) = 20. The probability of choosing a girl is 12/20 = 3/5.11. A bag contains 3 red balls and 4 blue balls. If two balls are drawn randomly without replacement, what is the probability that both balls are red? Answer: The total number of balls is 7. The probability of drawing the first red ball is 3/7. After one red ball is drawn, there are 2 red balls left and6 balls in total. So the probability of drawing the second red ball is 2/6. The overall probability is (3/7) * (2/6) = 1/7.12. There are 5 cards numbered from 1 to 5. Two cards are drawn randomly without replacement. What is the probability that the sum of the numbers on the two cards is even? Answer: There are two cases for the sum to be even. Either both cards are odd or both are even. There are 3 odd cards and 2 even cards. The probability of drawing two odd cards is (3/5) * (2/4) = 3/10. The probability of drawing two even cards is (2/5) * (1/4) = 1/10. So the total probability is 3/10 + 1/10 = 2/5.13. A box contains 6 white balls and 4 black balls. If three balls are drawn randomly with replacement, what is the probability that exactly two balls are white? Answer: The probability of drawing a white ball is 6/10 = 3/5. The probability of drawing a black ball is 4/10 = 2/5. Using the binomial probability formula, the probability of exactly two white balls is C(3,2) * (3/5)^2 * (2/5)^1 = 3 * 9/25 * 2/5 = 54/125.14. In a lottery, there are 10 tickets, and 3 of them are winning tickets. If two tickets are drawn randomly without replacement, what is the probability of getting at least one winning ticket? Answer: The probability of getting no winning tickets is (7/10) * (6/9) = 7/15. So the probability of getting at least one winning ticket is 1 - 7/15 = 8/15.15. There are 8 red marbles and 12 blue marbles in a bag. If four marbles are drawn randomly without replacement, what is the probabilitythat exactly two are red and two are blue? Answer: The total number of ways to draw four marbles is C(20,4). The number of ways to draw exactly two red and two blue marbles is C(8,2) * C(12,2). So the probability is [C(8,2) * C(12,2)] / C(20,4) = (28 * 66) / 4845 = 1848/4845.16. A box contains 5 red balls and 3 blue balls. If two balls are randomly drawn without replacement, what is the probability that both balls are red? Answer: The total number of ways to draw two balls is 8C2 = 28. The number of ways to draw two red balls is 5C2 = 10. So the probability is 10/28 = 5/14.17. There are 4 cards numbered 1, 2, 3, and 4. Two cards are randomly drawn without replacement. What is the probability that the sum of the numbers on the two cards is even? Answer: The total number of ways to draw two cards is 4C2 = 6. The ways to get an even sum are (1,3) and (2,4). So the probability is 2/6 = 1/3.18. In a bag of marbles, there are 6 green marbles and 4 yellow marbles. If three marbles are randomly drawn without replacement, what is the probability that exactly two are green? Answer: The total number of ways to draw three marbles is 10C3 = 120. The number of ways to draw exactly two green marbles is 6C2 * 4C1 = 15 * 4 = 60. So the probability is 60/120 = 1/2.19. A deck of cards has 52 cards. If two cards are randomly drawn without replacement, what is the probability that one is a heart and the otheris a spade? Answer: The number of ways to draw one heart and one spade is 13 * 13. The total number of ways to draw two cards is 52C2 = 1326. So the probability is (13 * 13)/1326 = 13/102.20. There are 7 boys and 5 girls in a class. If three students are randomly selected, what is the probability that two are boys and one is a girl? Answer: The total number of ways to select three students is 12C3 = 220. The number of ways to select two boys and one girl is 7C2 * 5C1 = 21 * 5 = 105. So the probability is 105/220 = 21/44.21. A box contains 5 red balls and 3 blue balls. If two balls are drawn randomly without replacement, what is the probability that both are red?Answer: The total number of balls is 8. The probability of drawing the first red ball is 5/8. After drawing one red ball, there are 4 red balls left and 7 balls in total. So the probability of drawing the second red ball is 4/7. The probability of both events happening is (5/8) * (4/7) = 5/14.22. In a class of 30 students, 15 are good at math and 20 are good at English. If a student is randomly selected, what is the probability that the student is good at both math and English?Answer: Let A be the event that a student is good at math and B be the event that a student is good at English. P(A) = 15/30 = 1/2 and P(B) = 20/30 = 2/3. Assuming the events are not mutually exclusive, P(A and B) = P(A) + P(B) - P(A or B). But we don't know P(A or B). However, we know that P(A or B) ≤ 1. So we can say P(A and B) ≥ P(A) + P(B) - 1 = 1/2 + 2/3 - 1= 1/6.23. A bag contains 4 green marbles and 6 yellow marbles. Two marbles are drawn one after the other without replacement. What is the probability that the first marble is green and the second is yellow?Answer: The probability of drawing a green marble first is 4/10. After drawing a green marble, there are 9 marbles left, 6 of which are yellow. So the probability of drawing a yellow marble next is 6/9. The probability of both events happening is (4/10) * (6/9) = 4/15.24. There are 10 books on a shelf, 4 of which are fiction and 6 of which are non-fiction. If two books are randomly selected without replacement, what is the probability that one is fiction and one is non-fiction?Answer: There are two ways this can happen: first fiction then non-fiction or first non-fiction then fiction. The probability of first fiction then non-fiction is (4/10) * (6/9) = 4/15. The probability of first non-fiction then fiction is (6/10) * (4/9) = 4/15. So the total probability is 4/15 + 4/15 = 8/15.25. In a survey, 80 people were asked if they like apples or oranges.50 people said they like apples and 40 people said they like oranges. If a person is randomly selected from this group, what is the probability that the person likes both apples and oranges?Answer: Let A be the event that a person likes apples and B be theevent that a person likes oranges. P(A) = 50/80 = 5/8 and P(B) = 40/80 = 1/2. Assuming the events are not mutually exclusive, P(A and B) = P(A) + P(B) - P(A or B). But we don't know P(A or B). However, we know that P(A or B) ≤ 1. So we can say P(A and B) ≥ P(A) + P(B) - 1 = 5/8 + 1/2 - 1 = 1/8.26. A bag contains 5 red balls and 3 blue balls. If two balls are drawn randomly without replacement, what is the probability that both are red?Solution: The total number of balls is 8. The probability of drawing the first red ball is 5/8. After drawing one red ball, there are 4 red balls left and 7 balls in total. So the probability of drawing a second red ball is 4/7. The probability of both events happening is (5/8) * (4/7) = 5/14.27. There are three boxes. Box A contains 2 red balls and 3 green balls. Box B contains 3 red balls and 2 green balls. Box C contains 4 red balls and 1 green ball. If a box is chosen at random and a ball is drawn from it, what is the probability that the ball is red?Solution: The probability of choosing each box is 1/3. The probability of drawing a red ball from box A is 2/5. From box B it's 3/5. From box C it's 4/5. So the overall probability is (1/3)*(2/5) + (1/3)*(3/5) + (1/3)*(4/5) = 3/5.28. In a lottery, there are 10 tickets numbered from 1 to 10. Two tickets are drawn randomly. What is the probability that the sum of the numbers on the two tickets is even?Solution: There are two cases for the sum to be even: both numbers are even or both numbers are odd. There are 5 even numbers and 5 odd numbers. The probability of drawing two even numbers is (5/10)*(4/9) = 2/9. The probability of drawing two odd numbers is (5/10)*(4/9) = 2/9. So the total probability is 2/9 + 2/9 = 4/9.29. A die is rolled twice. What is the probability that the sum of the two rolls is greater than 8?Solution: There are 36 possible outcomes when a die is rolled twice. The outcomes that sum to greater than 8 are (3,6), (4,5), (4,6), (5,4), (5,5), (5,6), (6,3), (6,4), (6,5), (6,6). That's 10 outcomes. So the probability is 10/36 = 5/18.30. There are four cards numbered 1, 2, 3, and 4. Two cards are drawn randomly without replacement. What is the probability that the product of the numbers on the two cards is even?Solution: The total number of ways to draw two cards is 4*3/2 = 6. The product will be even if at least one of the cards is even. There are three ways to draw one even card and one odd card (2 and 1, 2 and 3, 4 and 1, 4 and 3) and three ways to draw two even cards (2 and 4). So the probability is 6/6 = 1.。

概率论与数理统计试题（英文）09.1.8

概率论与数理统计试题（英⽂）09.1.82008~2009 第⼀学期《概率论与数理统计（Probability and Statistics ）》考试试卷（A 卷）（闭卷）Name Student I D Class Date: Jan 8, 2009 Time: 8:30 am to 11:00 amQuestion 1 (21 points) Filling the blanks (1). Let A , B be two events, P (A ) = 0.4，P (B ) = 0.3，P (B A ) = 0.6，then P (B ) = .(2). Suppose a random variable X has the probability distribution with20120.30.10.20.4-?? ???, then 2(1)P X ≥= . (3). Suppose ),(Y X has the joint density function<+>>=elsewherey x y x y x f ,01,0,0,2),(, then the marginal distribution for X alone )(x g = .(4). Suppose ),(~2σµN X ，consider a quadratic equation with one unknown220y y X ++= about y , if the probability of this equation having no real root is21, then µ= .(5). The number of distinct permutations can be made from the letters of the wordaccess = .∑==ni i X n X 11 , then =)(2X E . (7). Let n X X X ,,21 be a random sample of size n taken from population2(,)N µσ,µand 2σare unknown ,if 22[(),()]X k X k αα is a confidence interval for µ with confidence level α-1, then k = .(1). Suppose )1,0(~N X , )1,1(~N Y , if X and Y are independent, then ( )(A)2/1)0(=≤+Y X P(B) 2/1)1(=≤+Y X P (C) 2/1)0(=≤-Y X P (D) 2/1)1(=≤-Y X P(2). Suppose X ~ N ( 3, 4 )，Y ~ E ( 5 ) , find the error result ( )(A) ()8E X Y += (B) ()29D X Y +=(C) ()2263E X Y += (D) 50252X Y E ??+-=(3). Suppose that random variables X and Y are independent, if they have the sameprobability distribution with ???? ?3/23/121, then ==)(Y X P ( ) (A) 2/3 (B) 1 (C) 1/2 (D) 5/9(4). Suppose ),(~211σµN X , and ),(~222σµN Y , if 12(1)(1)P X P Y µµ-<>-<,then ( )(A) σ1<σ2(B) σ1>σ2 (C) µ1<µ2 (D) µ1>µ2Question 2 (18 points) Choosing the only one right result2(,)N µσ,∑=--=n i i X X n S 1221)(11，∑=-=n i i X X n S 1222)(1，∑=--=n i i X n S 1223)(11µ，∑=-=ni i X n S 1224)(1µ, then the following random variables which one is the t -distribution with n -1 degrees of freedom. ( ) (A) 11--n S X µ (B) 12--n S X µ (C) n S X 3µ- (D) n S X 4µ- (6). Suppose )(~λP X , 52+=X Y , then XY ρ= ( )(A) 1 (B) -1 (C) 1/2 (D) 0Suppose a random variable X has the density function ?<<=elsewhere x x x f ,010,2)(. Observe X independently for three times, let Y denote the number of an event }{21≤X occurring in three times, determine: (1) the probability distribution of Y ;(2) the probability distribution of 2Y .Question 3: (12 points)Suppose ),(Y X has the joint density function as follows:≤≤≤≤+=elsewhere y x y x c y x f 010,10)(),(2 . (1)Find the constant c ;(2) Are X and Y independent?(3) Determine the conditional distribution of X for any given value of Y . (4)11()22P X Y <=Question 4 (14 points)Suppose that a box contains one fair coin and one coin with a head on each side. Suppose also that one coin is selected at random and tossed, a head is obtained. Determine:(1) the probability of this event occurring ;(2) the probability that this coin is the fair coin when the above event occurred.Question 5 (10 points)There are 4 girls and 8 boys take part in interview of some company. Suppose that the interviewer select one student randomly every time, determine the probability that there are just 2 boys waiting for interviewing when all the girls have interviewed.Question 6 (5 points)Suppose a random variable X has the density function ??≤<-≤<-+=elsewhere x x x x x f 010,101,1)( ,let0,01,0X Y X0,1,X Z Xdetermine:(1) the joint probability distribution of (Y , Z ) ;(2) D(Y +Z ).Question 7 (12 points)Suppose a population has the density function <≥=--θθθx x e x f x ,0,3)()(3 , where θ is unknown parameter. Let n X X X ,,21 be a random sample of size n taken from population, find the maximum likelihood estimator for θ.Question 8 (8 points)。

中考英语概率计算单选题50题

中考英语概率计算单选题50题1. A box contains 5 red balls and 3 blue balls. If you randomly pick one ball, what is the probability of getting a red ball?A. 3/8B. 5/8C. 3/5D. 5/3答案：B。

本题考查概率的计算。

总共有8 个球，其中5 个是红球，所以抽到红球的概率是红球的数量除以总球数，即5÷8 = 5/8。

选项 A 是蓝球的概率；选项C 计算错误；选项D 不符合概率的取值范围。

2. You roll a dice. What is the probability of getting a number greater than 4?A. 1/6B. 1/3C. 1/2D. 2/3答案：B。

骰子一共有6 个面，数字大于4 的有5 和6 两个，所以概率是2÷6 = 1/3。

选项A 是单个数字出现的概率；选项C 是错误的；选项D 计算错误。

3. There are 10 cards numbered from 1 to 10. If you randomly draw one card, what is the probability of getting an even number?B. 1/5C. 2/5D. 4/5答案：A。

1 到10 中，偶数有2、4、6、8、10 共5 个，所以抽到偶数的概率是5÷10 = 1/2。

选项B 计算错误；选项C 不符合；选项D 也不对。

4. In a lottery, there are 20 tickets and only 5 of them are winning tickets. If you randomly choose one ticket, what is the probability of winning?A. 1/4B. 1/5C. 1/20D. 4/5答案：A。

高二英语概率计算单选题30题

高二英语概率计算单选题30题1. A box contains 5 red balls and 3 blue balls. If one ball is randomly drawn, the probability of getting a red ball is:A. 3/8B. 5/8C. 3/5D. 5/3答案：B。

本题考查概率的基本计算。

总球数为8 个，其中红球5 个，所以抽到红球的概率是5÷8 = 5/8。

选项A 是抽到蓝球的概率；选项C 计算错误；选项D 不符合概率的取值范围。

2. A fair coin is tossed twice. The probability of getting heads both times is:A. 1/2B. 1/4C. 1/3D. 2/3答案：B。

每次抛硬币得到正面的概率是1/2，两次都得到正面的概率是1/2×1/2 = 1/4。

选项 A 是单次抛硬币得到正面的概率；选项C 和D 计算错误。

3. In a deck of 52 cards, the probability of drawing a heart is:A. 1/4B. 1/13C. 1/52D. 4/13答案：A。

一副牌有52 张，其中红心有13 张，所以抽到红心的概率是13÷52 = 1/4。

选项B 是抽到特定点数的红心牌的概率；选项C 是抽到特定一张牌的概率；选项D 计算错误。

4. A bag has 2 green marbles and 4 red marbles. The probability of randomly picking a green marble is:A. 1/3B. 2/3C. 1/2D. 1/6答案：A。

总共有6 个弹珠，其中绿色2 个，所以抽到绿色弹珠的概率是2÷6 = 1/3。

选项B 是抽到红色弹珠的概率；选项C 计算错误；选项D 不符合计算结果。

中考英语概率计算单选题50题

中考英语概率计算单选题50题1.There are 10 balls in a box, 3 of them are red. What is the probability of drawing a red ball?A.1/10B.3/10C.7/10D.1/3答案：B。

解析：总共有10 个球，其中3 个是红色的，所以抽到红球的概率是红球的数量除以总球数，即3/10。

A 选项是抽到一个球的概率错误理解为1 除以总球数；C 选项是抽到非红球的概率；D 选项错误计算。

2.A coin is tossed. What is the probability of getting heads?A.1/2B.1/3C.1/4D.2/3答案：A。

解析：抛硬币只有正反两面，所以得到正面的概率是1/2。

B、C、D 选项错误理解抛硬币的概率。

3.There are 5 cards numbered from 1 to 5. What is the probability of drawing a card with number 3?A.1/5B.2/5C.3/5D.4/5答案：A。

解析：总共有5 张卡片，抽到数字3 的卡片只有1 张，所以概率是1/5。

B、C、D 选项错误计算抽到特定卡片的概率。

4.In a bag, there are 8 blue balls and 2 red balls. What is the probability of drawing a blue ball?A.1/5B.2/5C.4/5D.1/2答案：C。

解析：总共有8 个蓝球和2 个红球，总共10 个球，抽到蓝球的概率是蓝球数量除以总球数，即8/10 化简为4/5。

A、B、D 选项错误计算。

5.A box contains 6 apples and 4 oranges. What is the probability of picking an apple?A.1/10B.3/5C.2/5D.4/5答案：B。

中考英语概率计算单选题50题

中考英语概率计算单选题50题1.There are 10 balls in a box, 3 of them are red. What is the probability of drawing a red ball?A.1/3B.3/10C.7/10D.10/3答案：B。

本题考查概率的计算方法。

总共有10 个球，其中3 个是红色的，那么抽到红色球的概率就是红色球的数量除以总球数，即3/10。

选项A1/3 错误，因为不是这个比例。

选项C7/10 是抽到非红色球的概率。

选项D10/3 是错误的表达。

2.A bag contains 5 blue balls and 5 green balls. What is the probability of picking a blue ball?A.1/2B.1/5C.2/5D.5/2答案：A。

总共有 5 个蓝色球和 5 个绿色球，一共10 个球，那么抽到蓝色球的概率就是蓝色球的数量除以总球数，即5/10 化简为1/2。

选项B1/5 错误，不是这个比例。

选项C2/5 错误。

选项D5/2 是错误的表达。

3.There are 8 apples and 2 oranges in a basket. What is the probabilityof picking an orange?A.1/4B.1/5C.2/8D.8/2答案：A。

总共有8 个苹果和2 个橘子，一共10 个水果，那么抽到橘子的概率就是橘子的数量除以总水果数，即2/10 化简为1/5。

选项B1/5 错误，不是这个比例。

选项C2/8 错误。

选项D8/2 是错误的表达。

4.A box has 6 red marbles and 4 blue marbles. What is the probability of drawing a blue marble?A.2/5B.3/5C.4/6D.6/4答案：A。

中考英语概率计算练习题20题答案解析版

中考英语概率计算练习题20题答案解析版1. There are 5 red balls and 3 blue balls in a box. If you randomly pick one ball from the box, what is the probability of getting a red ball?A. 3/8B. 5/8C. 1/2D. 1/4答案：B。

解析：总共有5 + 3 = 8个球，红球有5个，根据古典概型概率公式，事件发生的概率等于该事件包含的基本结果数除以基本结果总数，所以拿到红球的概率是5÷8 = 5/8。

2. A bag contains 4 white marbles, 3 black marbles and 2 green marbles. What is the probability of drawing a black marble?A. 3/9B. 1/3C. 3/7D. 2/9答案：A。

解析：袋子里一共有4 + 3 + 2 = 9个弹珠，黑色弹珠有3个，按照概率公式，抽到黑色弹珠的概率为3÷9 = 3/9。

3. There are 10 lottery tickets, among which 2 are winning tickets. If you buy one lottery ticket randomly, what is the probability of winning?A. 1/10B. 1/5D. 1/2答案：B。

解析：总共有10张彩票，中奖的有2张，根据概率公式可得中奖概率为2÷10 = 1/5。

4. In a box, there are 6 yellow balls and 4 purple balls. What is the probability of taking out a purple ball?A. 2/5B. 3/5C. 4/10D. 6/10答案：A。

高二英语概率计算练习题40题含答案解析

高二英语概率计算练习题40题含答案解析1.A box contains 5 red balls and 3 blue balls. If you randomly pick one ball, what is the probability of getting a red ball?A.3/8B.5/8C.1/2D.2/3答案：B。

解析：总共有5 个红球和3 个蓝球，总共8 个球。

随机选一个球是红球的概率就是红球的数量除以总球数，即5 除以8 等于5/8。

2.There are 10 apples, 3 of which are bad. If you randomly choose one apple, what is the probability of getting a good apple?A.3/10B.7/10C.1/2D.4/5答案：B。

解析：总共有10 个苹果，3 个是坏的，那么好苹果有7 个。

随机选一个是好苹果的概率就是好苹果的数量除以总苹果数，即7 除以10 等于7/10。

3.A bag contains 4 green marbles and 6 yellow marbles. What is the probability of drawing a green marble?A.2/5B.4/10C.3/5D.2/3答案：A。

解析：总共有4 个绿色弹珠和6 个黄色弹珠，总共10 个弹珠。

抽到绿色弹珠的概率是绿色弹珠的数量除以总弹珠数，即4 除以10 等于2/5。

4.There are 12 students in a class. 5 students like math. What is the probability that a randomly chosen student likes math?A.5/12B.7/12C.1/2D.2/3答案：A。

解析：总共有12 个学生，5 个学生喜欢数学。