几何光学中的光线传输矩阵
几何光学中的光线传输矩阵高斯光束通过光学元件的变换

g1g2
0 g1g2 1
L
L
g1,2
1 2 f1,2
1
R1,2
rs为实数 rs Ce js C*e js
or
rs rmax sins
r0 rmax sin
r1 Ar0 B0 rmax sin
cos A D
2
rmax,
rs
n次往返传播矩阵:
Tn
1
sin
Asin n sinn 1
几何光学中的光线传输矩阵 (ABCD矩阵)
和
高斯光束通过光学元件的变换- ABCD公式
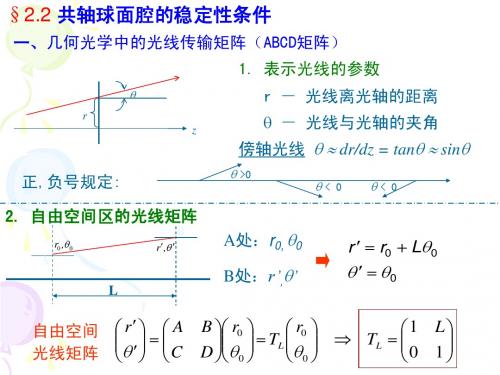
一、几何光学中的光线传输矩阵(ABCD矩阵)
r z
正,负号规定:
2. 自由空间区的光线矩阵
B
r0 ,0
r,
A
L
1. 表示光线的参数
r - 光线离光轴的距离 - 光线与光轴的夹角
傍轴光线 dr/dz = tan sin
L
1
B
L 2
L f2
C
1 f1
1 f2
1
L f1
D
L f1
1
L f1
1
L f2
rs1 Ars Bs
or
s
1 B
rs1
Ars
s1
1 B
rs2 Ars1
Crs Ds
1 B
rs2
Ars1
Crs
D B
rs1
Ars
rs2
2(
A
2
D
)rs
1
AD
BCrs
0
AD BC 1
rs2
A处 qA = q0+ l
C处 qc= qB+ lc
2-2腔稳定性条件
可见,同一谐振腔,不同 的传播次序,往返矩阵T不 相同,但(A+D)/2相同。
s
1
s 1
T1 T2
1 0 3 3 T1 T2 0 1 A D A D L 1 1 1,1 2 T1 2 T2 f 2 AD BC T1 AD BC T2 1
A处:r0, 0
A
r r0 L0
L
B处:r’,’
0
L 1
自由空间 光线矩阵
r A C
B r0 r0 1 TL TL 0 D 0 0
rs为实数 rs Ce js C *e js or r0 rmax sin A D cos 2
n次往返传播矩阵:
1 A sin n sinn 1 Tn C sin n sin
r1 Ar0 B 0 rmax sin
y
x
例:环形腔中的像散-对于“傍轴”光线
z
k1
f
k2
k3
对于平行于x,z平面传输的光线(子午光线),其 R cos 焦距 f x f cos 2 对于平行于“光轴”k和y确定的平面传输的光线 f R (弧矢光线),其焦距 fy cos 2 cos
二、共轴球面腔的稳定性条件——几何偏折损耗 1、稳定腔——傍轴光线在腔内任意多次往返不会横向逸出腔外
§2.2 共轴球面腔的稳定性条件
一、几何光学中的光线传输矩阵(ABCD矩阵)
1. 表示光线的参数
r z
r - 光线离光轴的距离 - 光线与光轴的夹角
第3讲_光线传输矩阵

– 程函(eikonal)方程:
– 光线的传播方向,就是程函 r 变化最快的方向 – 在讨论光线和几何光学的强度时,可以推导出光线的微分方程(光线方 程),其中 s 为光线上某点到另外一点的长度,而 r 是该点的位置矢量 :
2 r x y z
1 d r rS 1 d N ' 1 1 r ' r S 1 f1 f1 N
4.1 透镜波导光线稳定条件
综合可得到从S面到S+1面的光线传播情况
1 0 1 d 1 0 1 d r rS 1 1 S A B rS 1 ' ' ' 1 1 r 0 1 0 1 r C D r S 1 f1 S S f2
4.1 透镜波导光线稳定条件
• 双周期透镜波导的光线稳定条件 • 当θ 为实数时,光线与光轴的距离在rmax和-rmax之间振荡; 即光线传播被约束在透镜孔径形成的波导之中,不会发生 溢出。 • θ 为实数等价于|b|≤1,即:
d d d2 1 1 1 f1 f 2 2 f1 f 2
• 将矩阵形式的传播方程写成方程组的形式
1 rs ' (rs 1 Ars ) B
• 可得到递推关系
1 rs ' B (rs 1 Ars ) 1 rs 1 ' (rs 2 ArS 1) B r ' Cr Dr ' S S 1 S
' o
光线传输矩阵推导过程

光线传输矩阵推导过程光线传输矩阵是一种用于描述光线在光学系统中传输的数学工具。
它可以用来计算光线在光学系统中的传输路径和光强分布。
本文将介绍光线传输矩阵的推导过程。
我们需要了解一些基本概念。
在光学系统中,光线可以被描述为一条从一个点出发的矢量。
这个点可以是光源、物体或者像点。
光线的传输可以通过一系列的光学元件来实现,例如透镜、棱镜、反射镜等。
每个光学元件都有一个传输矩阵,它描述了光线在该元件中的传输过程。
假设我们有一个光学系统,由多个光学元件组成。
我们可以将整个系统看作是由多个小的光学元件组成的。
每个小的光学元件可以被描述为一个传输矩阵。
我们可以将这些小的传输矩阵组合起来,得到整个系统的传输矩阵。
现在,我们来推导一个光学元件的传输矩阵。
假设我们有一个光学元件,它将一个入射光线转换为一个出射光线。
我们可以将入射光线表示为一个列向量,出射光线表示为另一个列向量。
我们可以将这两个列向量组合成一个矩阵,称为传输矩阵。
传输矩阵的推导需要用到矩阵乘法的知识。
假设我们有一个光学元件,它将一个入射光线转换为一个出射光线。
我们可以将入射光线表示为一个列向量,出射光线表示为另一个列向量。
我们可以将这两个列向量组合成一个矩阵,称为传输矩阵。
假设我们有一个入射光线,它的方向向量为u,入射点为P1,出射点为P2。
我们可以将入射光线表示为一个列向量:u1 = [u1x, u1y, u1z, 0]T其中,T表示转置。
我们将最后一项设置为0,是因为我们只考虑光线的方向,而不考虑光线的位置。
同样地,我们可以将出射光线表示为一个列向量:u2 = [u2x, u2y, u2z, 0]T我们可以将光学元件的传输矩阵表示为一个4x4的矩阵M:M = [a, b, c, d;e, f, g, h;i, j, k, l;0, 0, 0, 1]其中,a、b、c、d、e、f、g、h、i、j、k、l都是实数。
我们可以将传输矩阵作用于入射光线上,得到出射光线:u2 = Mu1我们可以将这个式子展开,得到:u2x = au1x + bu1y + cu1z + du1wu2y = eu1x + fu1y + gu1z + hu1wu2z = iu1x + ju1y + ku1z + lu1wu2w = 0其中,w表示光线的强度。
光线转换矩阵

光线转换矩阵
一.光线的状态
光线的特征可以用其方向和线上一点的位置表示。
可用其相对于主光轴的角度表示其方向,
用线上一点到主光轴的距离表示该点的位置。
光线经过球面后,方向改变,上述角度和高度的数值
会发生改变。
二. 光线的矩阵表示
1.单球面的折射和反射
满足近轴条件,,,, ,
,注意,
,,为单球面的光焦度。
上述两式用矩阵表示,可写成
=。
其中=和=表示光线入射前后的状态,称为光线的
状态矩阵。
=表示折射球面的作用,称为折射矩阵。
对于反射球面,,
2.过渡矩阵
,
,为过渡空间的折射率;为过渡空间的长度。
=,=,称为过渡矩阵。
则, 。
为系统矩阵。
S为2×2矩阵,可表示为。
=
=
系统的光焦度
对于n个共轴球面系统,其系统矩阵一般可表示为。
三.成像矩阵的计算
=,=,
Q到P1处的过渡矩阵为
Pm到Q'处的过渡矩阵为
Q到Q'的光线的矩阵变换为
光线的变换用矩阵表示为
=
=
=
=
矩阵
=称为物像矩阵,
其行列式的值等于1。
近轴条件下,与无关,
(1)
物像矩阵化为
A=(2)
(3) 由(1)式可得
即 (4)
(4)式即为用物像矩阵元素表示的物像关系。
由(3)式可得系统的横向放大率为
(5)
由(2)可得,横向放大率亦可表示为
(6)
故系统的物像矩阵可记为
(7。
光线传输矩阵推导过程

光线传输矩阵推导过程光线传输矩阵(Ray Transfer Matrix)是描述光线在光学系统中传输的数学工具,也被称为 ABCD 矩阵。
在光学系统中,常常需要知道光线从一个位置传输到另一个位置,因此需要将光线传输过程用数学描述出来。
光线传输矩阵是一种简便的描述光线传输过程的方法,可以用于计算光学系统的成像性能、衍射现象等。
1. 光线传输是沿直线传播的;2. 光线的传播满足亥姆霍兹方程;3. 光学系统是轴对称的,即沿光路方向上的所有点都是轴对称的。
在这些假设的基础上,可以推导出光线传输矩阵的一般形式。
假设一束光线在一个点 P 处(位置矢量为 [x, y, z])的方向余弦分别为 l, m, n,那么在向前传播一段距离 d 之后,在点 Q(位置矢量为[x′, y′, z′])处的方向余弦分别为l′, m′, n′。
下面推导光线传输矩阵。
首先,根据第一条假设,可以得到:x′ = x + dly′ = y + dmz′ = z + dn然后,根据亥姆霍兹方程,可以得到:$$\frac{\partial^2\psi}{\partial x^2} + \frac{\partial^2\psi}{\partial y^2} + \frac{\partial^2\psi}{\partial z^2} + k^2\psi = 0$$其中,$\psi$ 表示复振幅,$k$ 表示波数,$k = 2\pi/\lambda$。
假设在点 P 处的复振幅为 $\psi_0$,则在点 Q 处的复振幅为:$$\psi = \psi_0e^{ikn′d}$$其中,$n′d$ 表示传输距离。
忽略高阶小量,可以进一步简化为:$$\frac{\partial^2\psi_0}{\partial x^2}dl^2 +\frac{\partial^2\psi_0}{\partial y^2}dm^2 + \frac{\partial^2\psi_0}{\partialz^2}dn^2 + 2\frac{\partial^2\psi_0}{\partial x\partial y}dldm +2\frac{\partial^2\psi_0}{\partial x\partial z}dldn +2\frac{\partial^2\psi_0}{\partial y\partial z}dmdn + k^2\psi_0 = 0$$接下来,定义一个 2x2 的矩阵 A 和一个 2x2 的矩阵 B,它们分别表示在点 P 和点Q 处的光线倾斜角(slopes)和射线高度(heights):然后,将光线传输方程改写为矩阵形式:$$\begin{bmatrix} l′ \\ m′ \end{bmatrix} = M\begin{bmatrix} l \\ m\end{bmatrix}$$其中,$M$ 表示光线传输矩阵,它可以通过将以上方程变形得到:根据矩阵乘法的定义,可以将 $M$ 表示为:其中,$$\begin{aligned} A_{11} &= 1 + \frac{\partial l}{\partial z}d +\frac{\partial^2\psi_0}{\partial x\partial z}d\frac{\partial l}{\partial z}d \\ A_{12} &= d + l\frac{\partial^2\psi_0}{\partial x\partial z}d \\ A_{21} &=\frac{\partial m}{\partial z}d + m\frac{\partial^2\psi_0}{\partial y\partial z}d \\ A_{22} &= 1 + \frac{\partial m}{\partial z}d + \frac{\partial^2\psi_0}{\partial y\partial z}d\frac{\partial m}{\partial z}d \\ B_1 &= x +l\frac{\partial\psi_0}{\partial z}d +\frac{1}{2}\left(\frac{\partial^2\psi_0}{\partial x^2}l^2 +\frac{\partial^2\psi_0}{\partial y\partial x}lm +\frac{\partial^2\psi_0}{\partial x\partial z}ldx\right)d \\ B_2 &= y +m\frac{\partial\psi_0}{\partial z}d +\frac{1}{2}\left(\frac{\partial^2\psi_0}{\partial y\partial x}lm +\frac{\partial^2\psi_0}{\partial y^2}m^2 + \frac{\partial^2\psi_0}{\partialy\partial z}mdy\right)d \\ C_1 &= -\frac{1}{2}\frac{\partial^2\psi_0}{\partial x\partial y}d^2lm \\ C_2 &= -\frac{1}{2}\frac{\partial^2\psi_0}{\partialy\partial x}d^2lm \end{aligned}$$可以看到,光线传输矩阵 $M$ 的每一项都可以用初始光线的方向余弦和位置来表示。
矩阵在几何光学中的应用

© 1995-2005 Tsinghua Tongfang Optical Disc Co., Ltd. All rights reserved.
工科物理 1998 年第 8 卷第 6 期 光学中的所有公式. 例如, 当光学系统为置于 空气 + k2 f ′ n′ 1
= (n ′ 1) 1 1
r1
17
总之, 利用矩阵方法, 的确可以很简便地 处理近轴几何光学中的主要问题. 当然, 这种 处理方法还可推广到非近轴几何光学中.
参 考 文 献
[ 1 ] 吴百诗 1 大学物理 1 西安: 西安交通大学出版
-
1
r2
+
n′ 1 1 l′ 1 n′ 1 r1 r2
这是几何光学中著名的透镜制造者方程.
nΑ 1 = nΑ x 1 = l Α+ x
1 传播矩阵与折射矩阵
在几何光学中, 借助于光线概念来研究 光在均匀介质中的传播或光通过某一光学系 统的传播及成像等问题. 利用矩阵作为数学 工具, 可以简化对上述问题的研究. 如图 1 所示, 设光线靠近光轴在折射率 为 n 的均匀介质中通过 P、 . 为了确定 Q 两点 光线的踪迹, 需要离光轴的横向位置 x 和光 线与光轴之间的夹角 Α这两个参量. 对于近 轴光线应有PQ ∆ l , 且
作了修改. 现将修改后的图刊登如下:
图 1 © 1995-2005 Tsinghua Tongfang Optical Disc Co., Ltd. All rights reserved.
n′ ′ 1Α 1 x′ 1
=
1
-
n′ n1 1 r1
n1Α 1 x1
( 6) ( 5)
n′ n′ 1- n1 2- n2 其中, k 1 = , k2= , 并且 n 2 = n ′ . 1 r1 r2
几何光学中的光线传输矩阵高斯光束通过光学元件的变换

A处:r0, 0 B处:r’,’
r r0 L0 0
自由空间 光线矩阵
r
A C
B D
r00
TL
r00
1 TL 0
L 1
3. 空气与介质(折射率为n2)的界面
r CA
入射 r0,0 出射 r,
B D
r00
Tn1n2
r00
n1 sin0 n2 sin '
n10
r
L f2
C
1 f1
1 f2
1
L f1
D
L f1
1
L f1
1
L f2
rs1 Ars Bs
or
s
1 B
rs1
Ars
s1
1 B
rs2 Ars1
Crs Ds
1 B
rs2
Ars1
Crs
D B
rs1
Ars
rs2
2(
A 2
D )rs1
AD
BCrs
0
AD BC 1
rs2
2(
A
2
D
)rs
2
f
f
可见,同一谐振腔,不同
的传播次序,往返矩阵T不
相同,但(A+D)/2相同。
s
1
s 1
T1 T2
T13
T23
1 0
0 1
A D
AD
1
L
1
1,1
2 T1
2 T2
f2
AD BC AD BC 1
T1
T2
思考题:
对1和2两种光线顺序, 分别求
rs rmax sins
2.0 ABCD光学矩阵

l1
环形腔
• 共轴球面镜腔 两反射镜为球面镜, 有共同光轴
凹面镜 R > 0; 凸面镜 R < 0; 平面镜 R=∞
• 稳定条件: 几何偏折损耗
稳定腔 任何傍轴光线可以在腔内往返无限多次不会 逸出腔外 几何偏折损耗小 (低损耗腔)
非稳定腔 傍轴光线有限次反射后便逸出腔外 几何偏折损耗大(高损耗腔)
1
f R 2
薄透镜与球面反射镜等效
5.ABCD矩阵的应用-球面镜腔的往返矩阵 球面镜腔中往返一周的光线矩阵(简称往返矩阵)
r11 TLTR2TLTR1 r00
T
r00
A C
B D
r00
T
A C
B D
1 2
R1
10
1 0
0 L
1 2
R2
10
1 0
0 L
A 1 2L R2
C
ቤተ መጻሕፍቲ ባይዱ
2 R1
2 R2
2
2
2
适用任何形式的腔,只需列出往返矩阵就能判断其稳定性
稳定判据另一表达式
A 1 2L R2
D
2L R1
1
2L R1
1
2L R2
1 A D 1 2L 2L 2L2
2
R1 R2 R1R2
1< 1 A D<1
2
0
<
1
L R1
1
L R2
<1
g1 1 L R1 g2 1 L R2
0 < g1g2 < 1
0 < g1g2 < 1或g1 0, g2 0 稳定腔;
g1g2 > 1, g1g2 < 0 非稳腔; • 只适用于简单的共轴 g1g2 0,g1g2 1 临界腔 球面镜腔(直腔)
光学设计总结知识点

光学设计总结知识点光学设计是一门综合性的学科,涉及光学原理、设计方法、软件应用等多个方面。
在光学设计中,掌握一些关键的知识点对于设计出高质量的光学系统至关重要。
本文将就光学设计的几个重要知识点进行总结,以帮助读者更好地理解和应用光学设计原理。
一、光学传输矩阵光学传输矩阵是光学设计中常用的一种数学工具,用于描述光线在光学系统中的传输规律。
光学传输矩阵能够将入射光线的位置、方向以及光线的传输路径等信息与出射光线的位置、方向等信息相联系。
通过光学传输矩阵,设计者可以快速计算光学系统中各个元件的参数以及光线的传输特性。
光学传输矩阵的计算方法多种多样,常见的有雅克比矩阵法、ABCD矩阵法等。
其中,ABCD矩阵法是最常用的一种方法,它基于光线的矢量表达,可用于描述球面透镜、薄透镜、光纤等光学元件的传输特性。
二、光学材料参数光学材料参数是指描述光学材料光学性质的一组参数,其中包括折射率、色散性质以及吸收性质等。
在光学设计中,准确地了解和使用光学材料参数是非常重要的。
不同的光学材料具有不同的折射率、色散性质和吸收性质,这些参数对于光学系统的设计和性能有重要影响。
折射率是光学材料重要的光学参数之一,它描述了光线在材料中的传播速度和传播方向的变化情况。
对于不同的波长和入射角,光的折射率一般是有变化的,因此在光学设计中需要考虑光学材料的色散性质。
三、光学设计软件光学设计软件是进行光学系统设计的重要工具,它能够帮助设计者进行光线追迹、光学优化以及系统性能分析等工作。
目前市场上存在着众多的光学设计软件,其中一些常用的有ZEMAX、CODE V、LightTools等。
在使用光学设计软件时,设计者需要了解软件的使用方法以及相关光学原理和设计原则。
只有熟练掌握光学设计软件的使用技巧,并结合光学设计的基本知识,才能更好地进行光学系统设计和优化工作。
四、光学系统的图像质量评价光学系统的图像质量评价是光学设计中的一个重要环节,它用于评估光学系统产生的图像质量是否满足设计要求。
2 光学谐振腔

光学谐振腔光学谐振腔是常用激光器的三个主要组成部分之一。
组成:在简单情况下,它是在激活物质两端适当地放置两个反射镜。
目的:就是通过了解谐振腔的特性,来正确设计和使用激光器的谐振腔,使激光器的输出光束特性达到应用的要求。
光学谐振腔的理论:近轴光线处理方法的几何光学理论、波动光学的衍射理论无源腔:又称为非激活腔或被动腔,即无激活介质存在的腔。
有源腔(激活腔或主动胺):当腔内充有工作介质并设有能源装置后。
一、构成、分类及作用1、谐振腔的构成和分类构成:最简单的光学谐振腔是在激光工作物质两端适当位置放置两个镀高反射膜的反射镜。
与微波腔相比光频腔的主要特点是:侧面敞开没有光学边界,以抑制振荡模式,并且它的轴向尺寸(腔长)远大于振荡波长:L》λ,一般也远大于横向尺寸即反射镜的线度。
因此,这类腔为开放式光学谐振腔,简称开腔。
开式谐振腔是最重要的结构形式----气体激光器、部分固体激光器谐振腔2、激光器中常见的谐振腔的形式1)平行平面镜腔。
由两块相距上、平行放置的平面反射镜构成2)双凹球面镜腔。
由两块相距为L,曲率半径分别为R1和R2的凹球面反射镜构成当R1=R2=L时,两凹面镜焦点在腔中心处重合,称为对称共焦球面镜腔;当R1+R2=L表示两凹面镜曲率中心在腔内重合,称为共心腔。
3)平面—凹面镜腔。
相距为L的一块平面反射镜和一块曲率半径为R的凹面反射镜构成。
当R=2L时,这种特殊的平凹腔称为半共焦腔4)特殊腔。
如由凸面反射镜构成的双凸腔、平凸腔、凹凸腔等,在某些特殊激光器中,需使用这类谐振腔5)其他形状的3、谐振腔的作用(1) 提供光学正反馈作用谐振腔为腔内光线提供反馈,使光多次通过腔工作物质,不断地被放大,形成往复持续的光频振荡;取决因素:组成腔的两个反射镜面的反射率,反射率越高,反馈能力越强;反射镜的几何形状以及它们之间的组合方式。
上述因素的变化会引起光学反馈作用大小的变化,即引起腔内光束能量损耗的变化。
(2) 对振荡光束的控制作用主要在方向和频率的限制,其功能为:①有效地控制腔内实际振荡的模式数目,使大量的光子集结在少数几个沿轴向、且满足往返一次位相变化为2π的整数倍的光子状态中,提高了光子简并度,从而获得单色性好、方向性好及相干性强的优异辐射光。
abcd光学矩阵计算

abcd光学矩阵计算光学矩阵是光学系统中的一种重要工具,能够用来描述光线通过光学系统时的传播和变换规律。
其中,abcd矩阵是一种常用的光学矩阵,用来描述光线通过光学元件时的光学行为。
abcd矩阵是一个2×2的矩阵,表示光线的传输和变换过程。
矩阵的元素a、b、c、d分别代表了光线的传输系数和变换系数。
通过计算abcd矩阵,可以得到光线通过光学元件后的位置和方向的变化关系。
在光学系统中,光线的传输可以通过矩阵乘法来描述。
假设有一个光学元件,其光学矩阵为M,光线的入射位置和方向分别为(x, θ),则光线的出射位置和方向可以通过以下公式来计算:(x', θ') = M * (x, θ)其中,(x', θ')为光线的出射位置和方向,M为光学矩阵,(x, θ)为光线的入射位置和方向。
在实际应用中,光学系统通常由多个光学元件组成。
假设光学系统由n个光学元件组成,其光学矩阵分别为M1、M2、...、Mn,光线的入射位置和方向为(x, θ),则光线的出射位置和方向可以通过以下公式来计算:(x', θ') = Mn * ... * M2 * M1 * (x, θ)通过以上公式,我们可以计算出光线在整个光学系统中的传输和变换过程。
这对于光学系统的设计和分析非常重要。
需要注意的是,abcd矩阵描述的是近轴光线的传输和变换过程。
对于大角度入射的光线,abcd矩阵的应用会有一定的限制。
此外,abcd矩阵的计算也需要考虑光学元件的非理想性,如光学元件的形状误差、材料非均匀性等因素。
在实际应用中,光学矩阵的计算可以通过多种方法实现。
一种常用的方法是使用矩阵乘法和矩阵求逆的操作。
通过将光学元件的传输和变换关系表示为矩阵形式,并进行矩阵运算,可以得到光学矩阵的结果。
除了abcd矩阵,还有其他表示光线传输和变换的方法,如传输矩阵法和物方传输函数法等。
这些方法在不同的光学系统分析和设计中有着各自的应用。
光线转换矩阵

物像矩阵: A = T S T
d e t A=1
x x xx x y ( S 21 S 22 S11 S12 )n11 ( S 22 S12 ) y n1 nm n1nm nm
在近轴条件时,y´与α1 应无关系,即自物 点发出的所有光线经系统成像后都会聚于对 应的像点上。
2 1 对于反射镜: n n 1, r f 1 1/ f R 0 1
二、过渡矩阵和系统矩阵
若一个光学系统由两个相邻的共轴单 球面组成,d21 为两单球面之间的距离。 入射光线 r1 出射光线 r 2 ´
P1 和 P2 的状态分别为 r1´和 r2
Q处的光线经过系统到 Q´时的矩阵转换, 只需按光线进行的前后将这两过渡矩阵依次 作用于系统矩阵,即
TQm ST1Q rQ rQ
m 1 0 S11 S12 1 0 n11 nm y y S S x / n 1 x / n 1 m 21 22 1 0 S11 ( x / n1 ) S12 S12 n11 1 y S ( x / n )S S x / n 1 m 1 22 22 21 S11 ( x / n1 ) S12 S12 n11 y S ( x / n ) S ( x / n ) S xx /( n n ) S S x / n S 1 22 m 11 1 m 12 22 m 12 21
S12
厚透镜:透镜的厚度 d21 不可忽略
S R2T21R1
0 1 1 1 2 1 0 1 0 1 d / n 1 21 1 1 2 1 0 1 d / n d / n 1 21 1 21 1 1d 21 / n 1 1 2 d 21 / n 2 d /n d / n 1 21 1 21
2.0 ABCD光学矩阵

An, Bn, Cn, Dn 矩阵元有界,说明光线经过n次不逸出
腔外,关键因子 应为实数,且不等于kp
讨论: arccos1 A D
2
(1)
1 A D < 1
2
实数,
An, Bn, Cn, Dn 有界 稳定腔
(2)
1 A D > 1
2
复数, nAn, Bn, Cn, Dn 非稳定腔
1
2L R1
B
2L1
L R2
D
2L R1
1
2L R1
1
2L R2
往返n次的光线矩阵
rnn
T
T
n
r00
Tn
r00
An Cn
Bn Dn
r00
Tn
1
sin
Asin
n
C
sin n
sin n
1
其中
arccos
1 2
A
D
Dsin
Bsin n
n sin n
1
• 光线在谐振腔中的传输可等效为在透镜波导中的传输。
• 往返矩阵与初始坐标、出发位置及往返顺序无关。
• 谐振腔往返矩阵的建立方法:等效透镜波导;确定 一个周期;写出各部分光线矩阵的乘积。
球面镜腔可以等效为周期透镜波导
L
L
R1
R2
R1
复杂腔的等效周期透镜波导及往返矩阵
l3
l2
l1 l2 l3
l1 l2 l3
l1
10
l1 1
1 1
f1
10 10
l2 1
1 1
f
2
10 10
l3 1
1 1
高斯光束的传输变换

U 00 (x, y, z)
=
c
−ik r 2 [ 1 −i λ ]
e e 2 R( z) πw2 ( z) −i(kz+Φ)
w( z )
(2.7.15)
定义一个新的复参数 q(z)
1 = 1 −i λ q(z) R(z) πw2 (z)
任一旁轴光线在某一给定参考面内都可以由两个坐标参数来表征,光线离轴线的距离 r 及光线与轴线的夹角θ。将这两个参数构成一个列阵,各种光学元件或光学系统对光线的变 换作用可用一个二行二列的方阵来表示,变换后的光线参数可写成方阵与列阵乘积的形式。 1. 近轴光线通过距离 L 均匀空间的变换
我们分析近轴光线在均匀空间通过距离L的传输,如图 2-22 所示,假定光线从入射参考 面P1出发,其初始坐标参数为r1和θ1,传输到参考面P2时,光束参数变为r2和θ2,由几何光 学的直进原理可知
图 2-26 高斯光束的聚焦 由前面介绍,光线从入射光束束腰处传输到出射光束束腰处的传输矩阵
⎜⎜⎝⎛ CA
DB ⎟⎟⎠⎞ = ⎜⎜⎝⎛10
1l'⎟⎟⎠⎞⎜⎜⎝⎛
−
1 1/
F
10⎟⎟⎠⎞⎜⎜⎝⎛
1 0
1l ⎟⎟⎠⎞ = ⎜⎜⎝⎛1−−1l/' /FF
l
+ 1
l'−ll' / −l/F
F
⎟⎟⎠⎞
(2.7.26)
w0 ' ≈ w0π
λF
= λF , l'≈ F
1 + (λl / πw02 )2 πw(l)
(2.7.32)
几何多光束和矩阵式

几何多光束和矩阵式
几何多光束和矩阵式是一种计算光路的方法。
它适用于特定的光学系统,如激光器、光纤通信和成像系统等。
该方法将光束的传播视为矩阵操作,使得对于复杂的光路结构能够进行有效的计算和分析。
在几何多光束和矩阵式中,光线被描述为一个光束矩阵,其中包含光线的位置和方向信息。
通过将光束矩阵和光学元件的传输矩阵相乘,可以计算出光束在光学系统中的传输过程。
这种方法非常适用于复杂的光学系统,因为只需要计算每个光学元件的传输矩阵,就可以得出整个系统的传输矩阵。
几何多光束和矩阵式不仅适用于传统的几何光学,也适用于波动光学。
在波动光学中,光束被描述为具有振幅和相位的波,而光学元件的传输矩阵被表示为复数形式的传输函数。
通过将光束的振幅和相位信息编码为矩阵,波动光学系统的传输过程也可以用矩阵相乘的形式表示。
总之,几何多光束和矩阵式是一种非常有效的计算光路的方法,尤其适用于复杂的光学系统。
通过将光线和波的传输过程描述为矩阵操作,可以简化计算过程,提高计算效率,为光学系统设计和分析提供了更多的工具和方法。
- 1 -。
第三章激光原理光学谐振腔理论(ABCD矩阵)

Tn1n2
r00
n1 sin0 n2 sin '
n10
r
r0
n2
n1 n2
0
1 0 Tn1n2 0 n1 n2
4. 薄透镜传输矩阵
r, r,
r r r l r l
腔内光子
平均寿命
t
1 N0
dN t
1 N0
t 0
N0
R
e
t R
dt
t
td
e
R
0
R
•谐振腔损耗越小,腔内光子寿命越长
•腔内有增益介质,使谐振腔净损耗减小,光子寿命变长
3、光子寿命与无源谐振腔的Q值的联系
定义: Q 储存在腔内的总能量(E)
二、腔的模式
腔的模式:光学谐振腔内可能存在的电磁场的本征态
谐振腔所约束的一定空间内存在的电磁场,只能存在
于一系列分立的本征态
腔内电磁场的本征态
麦克斯韦方程组 腔的边界条件
因此:
腔的具体结构 腔内可能存在的模式(电磁场本征态)
模的基本特征主要包括: 1、每一个模的电磁场分布 E(x,y,z),腔的横截面内的 场分布(横模)和纵向场分布(纵模);
非选择损耗 (无 选模作用)
腔内损耗的描述—— 平均单程损耗因子
定义无源腔内,初始光强I0往返一次后光腔衰减为I1,则
I1 I0e2
1 ln I0
I0
2 I1
I1
对于由多种因素引起的损耗,总的损耗因子可由各损耗因子相
光学成象中的矩阵方法(i)──光线变换矩阵和abcd定理

光学成象中的矩阵方法(i)──光线变换矩阵和abcd定理
光学成象中的矩阵方法是一种用于研究和描述光线变换的数学工具,可广泛应用于光学和光学成象的研究。
有两种主要的光线变换矩阵方法:光线变换矩阵和abcd定理。
光线变换矩阵(Ray Tracing Matrix)用于描述从一个场景中光线如何传播到另一个地方。
它是一种矩阵数学,可以用于分析特定光线的变换情况,也可以用于阐释光线与物体表面形式的响应。
它的应用主要有两个阶段:追踪和折射。
追踪的过程称为折射,它描述了光线从光源发射到目标处产生折射的情况。
而折射的过程则描述了光线从目标物体表面穿过时,在反射、折射和其他方面的变化。
另一种矩阵方法是abcd定理,即“旋转平板-棱镜-折射矩阵定理”。
它是一个描述光线入射光路和出射光路之间变换关系的理论,是一种把在不同环境中发生的光学成象结果用矩阵来描述的方法。
abcd定理是一种具有双子性的矩阵理论,能使用户通过建立一个矩阵来更好的理解发生在不同环境中的光学成象结果。
通过光线变换矩阵和abcd定理,可以在简单和精确的方式中分析光线的变换情况,进而帮助用户更快速的了解物体的表面形状和准确的传播光线。
两种矩阵方法都特别有用,在光学研究和光学成象中发挥着重要作用,不仅有助于快速高效地分析和理解光线变换,而且还可以有效地准确预测出物体表面变化所产生的光学成象结果。
因此,光线变换矩阵和abcd定理已经成为光学和光学成象研究中不可替代的数学技术手段。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
1
r3
3
2 R2
0
1
r2
2
TR2
r2
2
当光线再从镜M2行进到镜M1面上时
r4
4
1 0
L
1
r3
3
TL
r3
3
然后又在M1上发生反射
• Ray optics and geometrical optics in fact contain exactly the same physical content, expressed in different fashion.
• Ray matrices or “ABCD matrices” are widely used to describe the propagation of geometrical optical rays through paraxial optical elements, such lenses, curved mirrors, and “ducts”. These ray matrices also turn out to be very useful for describing a large number of other optical beam and resonator problems, including even problems that involve the diffractive nature of light.
1 0 1 0
TR
2 R
1
1 f
1
球面镜对傍轴光线的反射变换与焦距为f=R/2的 薄透镜对同一光线的透射变换是等效的。
用一个列矩阵描述任一光线的坐标,用一个二 阶方阵描述入射光线和出射光线的坐标变换。
该矩阵称为光学系统对光 线的变换矩阵。
r2
2
A C
B r1
D
1
• Ray optics---by which we mean the geometrical laws for optical ray propagation, without including diffraction---is a topic that is not only important in its own right, but also very useful in understanding the full diffractive propagation of light waves in optical resonators and beams.
• Ray matrices or paraxial ray optics provide a general way of expressing the elementary lens laws of geometrical optics, or of spherical-wave optics, leaving out higher-order aberrations, in a form that many people find clearer and more convenient.
二 腔内光线往返传播的矩阵表示
• 由曲率半径为R1和R2的两个球面镜M1和M2组 成的共轴球面腔,腔长为L,开始时光线从M1 面上出发,向M2方向行进
当凹面镜向着腔内时,R取正值;当凸面镜向 着腔内时,R取负值
光线从M1面上出发到达
L
1
r1
1
TL
r1
1
当光线在曲率半径为R2的镜M2上反射时
• Ray propagation through cascaded elements:
• A single 4-element ray matrix equal to the ordinary matrix product of the individual ray matrices can thus describe the total or overall ray propagation through a complicated sequence of cascaded optical elements. Note, however, that the matrices must be arranged in inverse order from the order in which the ray physically encounters the corresponding elements.
一 光线传输矩阵
• 腔内任一傍轴光线在某一给定的横截面内都 可以由两个坐标参数来表征:光线离轴线的
距离r、光线与轴线的夹角。规定:光线出 射方向在腔轴线的上方时, 为正;反之,
为负。
• 光线在自由空间行进距离L时所引起的坐标
变换为
1 L
TL 0
1
球面镜对傍轴光线的 变换矩阵为(R为球 面镜的曲率半径)
• Since a ray is, by definition, normal to the optical wavefront, an understanding of the ray behavior makes it possible to trace the evolution of optical waves when they are passing through various optical elements. We find that the passage of a ray (or its reflection) through these elements can be described by simple 2x2 matrices. Furthermore, these matrices will be found to describe the propagation of spherical waves and of Gaussian beams such as those which are characteristics of the output of lasers.
