静电场的微分方程与解的唯一性(双语)
边值问题和唯一性定理(静电场)

静电场的边值问题
静电场的唯一性定律
目前可解决的静电场问题
电荷在有限区域内,电荷的分布情况已知,并 且介质为线性各向同性均匀介质中的静电场问 题。对于此类问题,一般可以先求出电位,再 计算场中各点的电场强度和电位移矢量。 电荷、介质分布具有某种对称性的问题。由于 电荷和介质的分布具有对称性,因此电位移矢 量的分布必然也具有对称性。在这种情况下, 可以先用高斯通量定理求解电位移矢量,然后 再求电场强度。 已知电场的分布求电荷分布的问题。在这种情 况下,可直接由公式计算电荷的体密度,导体 上的面电荷密度根据分界面条件确定。
2
静电场边值问题的提出
实际中对于很多电磁场的问题通常并不 知道电荷分布,如静电场中导体表面的 感应电荷分布,介质极化后极化电荷的 分布等。对于此类的问题,必须通过求 解满足给定边界条件的电位微分方程 (泊松方程或拉普拉斯方程)的电位函 数,进而再求场域中的电场强度。我们 把这种在给定边界条件下,求解泊松方 程或拉普拉斯方程的问题称为边值问题。
对于各向同性、线性的非均匀媒质,电位 满足的微分方程又是什么形式呢?
D
D E
E
( )
7
边值问题举例-直接积分法
例 设有电荷均匀分布在半径为a的介质球型区域中,电荷 体密度为 ,试用解微分方程的方法求球体内、外的电位 及电场。(同例2-4) 解:采用球坐标系,分区域建立方程
自学)
10
反设满足场的解答有两个相异的解答1和 2,则差
场u= 1 2 满足拉普拉斯方程
2 2
u 1 2 0 根据矢量恒等式
chapter2-2 静电场的唯一性定理-2015-09-28

(2.2)
至此,对于区域 V 而言,我们还不知道外边界上 的条件。这个问题正是唯一性定理所要解决的:就是 我们还需要知道外边界上的什么条件之后,求能够唯 一确定区域内的静电场。 2)唯一性定理的内容:若
i)区域 V 内给定自由电荷分布 f x ; ii)区域的外边界 S 上给定电势 S , 或者电势的法向导数 n ,
唯一性定理定理也表明, a)唯一性定理对于静电问题的重要性在于:只要我 们得到一个满足泊松方程以及相应的边界条件的
解,那么这个解一定就是该问题的严格解。 b)从方法论上,我们根据物理直觉和物理图像可以 猜测出一些问题的解,此时唯一性定理保证了其 正确性 c)如果我们针对这类边值问题, 找到一个试探的解, 但若我们验证这个试探的解满足上述的几个条件, 包括验证它是否满足微分方程,是否满足内部的 边值关系,以及在外边界上是否满足边值关系, 如果都满足,那这个试探解就是这个问题的解; d)有时,我们在给出一个试探解的时候,可以在一 开始保留 1-2 个未知的系数(但并不影响所满足 的微分方程) , 然后根据边值关系, 来确定这些系 数。 2、有导体存在时的唯一性定理 对于导体存在的静电问题,每个导体上的总电荷 Q 与电势φ实际上是一对共轭量, 通常求解这类问题时不 可能同时预先设定每个导体上的总电荷和电势。 因此,当有导体存在时,为了确定电场,我们可以 根据这一对共轭量,将导体的静电问题设置为以下两 类问题: 第一类问题:给定每个导体上的电势 i ;
f x ;
b)在 V 的外边界 S 上给定 S ,或者电势的法向导数
n S ;
c) 势 i 亦给定, 则 V ' 内的电场唯一确定。
每个导体 i 的电
由于当给定了导体的电势后相当于给定了体系完 备的外边界条件,那么给定导体的唯一性定理就退化 成了一般形式,因此此定理的证明方法同上。 2)第二类问题的唯一性定理:
静电场的微分方程与解的唯一性(中文)

通解。
数学物理方程描述物理量随时间和空间的变化特性。
定解条件
初始条件 边界条件
静电场与时间无关,因此电位所满足的泊松方程及
拉普拉斯方程的解仅决定于边界条件。
根据给定的边界条件求解空间任一点的电位就是静
电场的边值问题。
此处边界条件实际上是指给定的边值,它不同 于前一章描述静电场的边界上场量变化的边界条件。
第三章 静电场的边值问题
主要内容 电位微分方程、镜像法、分离变量法。
1. 电位微分方程 2. 镜像法 3. 直角坐标系中的分离变量法 4. 圆柱坐标系中的分离变量法 5. 球坐标系中的分离变量法
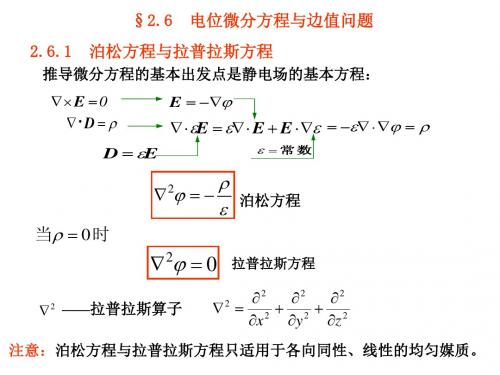
1. 电位微分方程 已知电位 与电场强度 E 的关系为
E
对上式两边取散度,得
EHale Waihona Puke 2对于线性各向同性的均匀介质,电场强度 E
的散度为
E
那么,电位满足的微分方程式为
2
泊松方程
2
对于无源区, ,0 上式变为
2 0
拉普拉斯方程
已知分布在 V 中的电荷 (r在) 无限大的自由空
间产生的电位为
(r)
1 4π
(r) dV V| r r|
上式为泊松方程在自由空间的特解。
利用格林函数可以求出泊松方程在有限空间的
可以证明电位微分方程解具有惟一性。
若静电场的边界为导体,此时给定导体上的电位就
是第一类边界。 已知
ᄊ ᄊn
S
可见,表面电荷给定等于给定了电位的法向导数值。
因此,若给定导体表面上的电荷量就是第二类边界。
因此,对于导体边界,当边界上的电位,或电位 的法向导数给定时,或导体表面电荷给定时,空间的 静电场即被惟一地确定。这个结论称为静电场惟一性 定理。
第3章静态场的边值问题及解的唯一性定理

r
O
d
P(r, ) R q
处理方法:电位叠加原理:
1、先假设导体球面接地,则球面上存在电量为q' 的感应电荷, 镜像电荷可采用前面的方法确定q a q, d。 a2 2、为了满足电荷守恒原理。断开接地d线,将电d量为-q'的电荷加 到导体球面上,使这些电荷均匀分布在球面上,使导体球为等势 体,且表面总电荷为零。
l′
2 x2 (z h)2
均匀带电直线的电位分布
z 0,R R z0 0
l ln R C l ln R0
2
2 R
显然,满足边界条件。所以,原问题不变,所得的解是正确的。
11
第3 章
例3. 点电荷对相交半无限大接地导体平面的镜像 如图所示,两个相互垂直相连的半无限大接地导体平板,点
像把导体平面抽走一样,用两点电荷的场叠加计算。
7
第3 章
解:用一个处于镜像位置的点电荷代替导体边界的影响, 则z>0空间任一点 P 的电位由 q 及 q' 共同产生,即
q q 1 (
q
q
)
4π0 r 4π0 r 4π0 x2 y2 (z h)
x2 y2 (z h)2
l
2π
r r er
以r 0 为参考点,则电位
r r0
Edr
l 2π
ln
r0 r
l 2π
ln
1 r
C
1)长直线电荷与接地的长直圆柱导体平行,求圆柱外电位分布
在圆柱与线电荷之间,在圆柱内离轴线的距离b 处,平行放置一
根镜像线电荷 , 代替圆柱导体上的感应电荷.
2.6 静电场边值问题 唯一性定理

V/m
CQU
2.6.3 唯一性定理
1、唯一性定理 在静电场中满足给定边界条件的电位微分方程 满足给定边界条件的电位微分方程( 在静电场中满足给定边界条件的电位微分方程(泊松方 程或拉普拉斯方程)的解是唯一的, 程或拉普拉斯方程)的解是唯一的,称之为静电场的唯一性定 理。 2. 唯一性定理的重要意义 可判断静电场问题的解的正确性 解的正确性: • 可判断静电场问题的解的正确性: 唯一性定理为静电场问题的多种解法(试探解、数值解、 • 唯一性定理为静电场问题的多种解法(试探解、数值解、 解析解等)提供了思路及理论根据。 解析解等)提供了思路及理论根据。
S
第三类 边界条件
(ϕ + β ∂ϕ ) = f3 ( s) ∂n S
第四类 边界条件
ϕ S = f1 ( s)
求解边值问题注意事项: 求解边值问题注意事项:
CQU
点电荷的场
1.根据求解场域内是否有 ρ 存在,决定电位满足泊松方程还是拉氏 .根据求解场域 求解场域内是否有 存在,决定电位满足泊松方程还是拉氏 泊松方程还是 方程,然后判断场域是否具有对称性,以便选择适当的坐标系。 方程,然后判断场域是否具有对称性,以便选择适当的坐标系。 2.正确表达边界条件,并利用它们确定通解的待定常数。 正确表达边界条件,并利用它们确定通解的待定常数。 3.若所求解的场域内有两个(或以上)的均匀介质区域,应分区求 若所求解的场域内有两个(或以上)的均匀介质区域, 分区求 场域内有两个 不能用一个电位函数表达两个区域的情况。这时会出现4 解。不能用一个电位函数表达两个区域的情况。这时会出现4个积分 常数,还需考虑介质分界面上的衔接条件来确定积分常数。 分界面上的衔接条件来确定积分常数 常数,还需考虑介质分界面上的衔接条件来确定积分常数。 4.对于开域问题,还需给出无限远处的自然边界条件。 4.对于开域问题,还需给出无限远处的自然边界条件。当场域有 对于开域问题 限分布时,应有: 限分布时,应有:
§26 静电场边值问题 唯一性定理
2
2
x 2
2
y 2
0
(阴影区域)
( xb,0 yb及yb,0xb) U0
图 2.6.4 缆心为正方形的同轴电缆横截面
0 x2 y2 a2 ,x0, y0
x
0 ( x0,b ya)
y
0 ( y 0,b xa )
图 2.6.5 体电荷分布的球形域电场
积分得通解
1(r)
r2 6 0
C1
1 r
C2
2 (r)
C3 r
C4
边界条件
1 ra 2 ra
1 r0 有 限 值
0
1
r
ra
0
2
r
ra
2 r 0 参考点电位
解得 电位:
C1 0 C4 0
对场域求体积分,并利用高斯散度定理
(uu)dV uu dS (u)2dV
V
s
V
S为体积V的边界面,即S S0 S,S S1 S2 Sn , 由于在无穷远S0处电位为零,因此有
图2.6.6 证明唯一性定理用图
uu dS uu dS (u)2 dV
C3
a2 2 0
,
C2
a3 3 0
1(r)
6 0
(3a 2
r2)
2 (r)
a3 3 0 r
电场强度(球坐标梯度公式):
E1 (r )
1
1
r
er
r 3 0
er
静电场边值问题的唯一性定理

静电场边值问题的唯一性定理摘要:静电场边值问题及其唯一性定理是一重要知识点,定理的表述和证明都涉及较多的数学知识。
由于唯一性定理的概念对于许多问题(如静电屏蔽)的确切理解有很大帮助,所以我们将给此定理一个物理上的论证,期待大家能从中有所受益. 关键词:静电场;边值;唯一性;静电屏蔽1、问题的提出实际中提出的静电学问题,大多不是已知电荷分布求电场分布,而是通过一定的电极来控制或实现某种电场分布。
这里问题的出发点(已知的前提),除给定各带电体的几何形状、相互位置外,往往是在给定下列条件之一;(1) 每个导体的电势U K ; (2) 每个导体上的总能量Q K ;其中K=1,2,……为导体的编号。
寻求的答案则是在上述条件(称为边界条件)下电场的恒定分布。
这类问题称为静电场的边值问题。
这里不谈静电场边值问题如何解决,而我们要问:给定一组边界条件,空间能否存在不同的恒定电场分布?唯一性定理对此的回答是否定的,换句话说,定理宣称:边界条件可将空间里电场的恒定分布唯一地确定下来。
2、几个引理在证明唯一性定理之前,先作些准备工作——证明几个引理。
为简单起见,我们暂把研究的问题限定为一组导体,除此之外的空间里没有电荷。
(1)引理一 在无电荷的空间里电势不可能有极大值和极小值。
用反证法。
设电势U 在空间某点P 极大,则在P 点周围的所有邻近点上梯度U ∇ρ必都指向P 点,即场强U E ∇-=ρρ的方向都是背离P 点的(见图1-1a 。
)这时若我们作一个很小的闭合面S 把P 点包围起来,穿过S 的电通量为0)(>⋅=⎰S d E S E ρρϕ (1)根据高斯定理,S 面内必然包含正电荷。
然而这违背了我们的前提。
因此,U 不可能有极大值。
用同样的方法可以证明,U 不可能有极小值(参见图1-1b )。
(2)引理二 若所有导体的电势为0,则导体以外空间的电势处处为0。
因为电势在无电荷空间里的分布是连续变化的,若空间有电势大于0(或小于0)的点,而边界上又处处等于0,在空间必然出现电势的极大(或极小)值,这违背引理一。
电动力学uniquenesstheorem唯一性定理完全解读

引入标量函数Φ ,令Φ = '- ″
2 , 2 , 2 0
i
i
在区域边界面S 上
S
S
0 S
(给定第一类边界条件)
或 ,
n S n S
0
n S
(给定第二类边界条件)
下面需要证明旳是,满足以上方程和边界条件旳'和
1) 绝缘介质静电问题旳唯一性定理及证明 在有限旳边界区域V 内有几种均匀旳绝缘介质Vi 、εi
(i = 1、2、3 …) ,V 中旳自由电荷分布(ρ或σ) 为已知,那
么,当V 旳边界面S 上旳电势 给 定(或电势旳法向导数边
界条件) ,则V 内旳电场有唯一拟定旳解。
数学表述如下:
2 i
i
(在每个小区Vi)
V′旳全部内、外表面上都有一定旳值或 值,应用有关绝缘介
质旳唯一性定理,则V′内旳电场必有唯一解. n
b)区域V 内有若干导体,假设除导体以外旳区域V′内旳自由电荷分
布ρ已知,V′旳外表面S 上有已知旳值或 值,另外,若每个导
n 体所带旳总电量Qi 为已知,则区域V′内旳电场有唯一解。
数学表达为:
场有唯一解。这么,有导体存在时静电问题旳唯一性定理 也得到证明。
最终需要强调一点,尽管唯一性定理并不给出求解泊松方程旳详细措 施与环节,但它对于处理实际旳边值问题有着主要旳意义. 首先,它明 确了在哪些条件下能够唯一地拟定一种静电场,即给出了求解静电场 旳根据;其次,它使我们能够灵活地选用最简朴、最合适旳解题措施, 甚至能够猜一种解(即提出尝试解) . 只要这个解确实满足了问题中 旳场方程和全部定解条件,那么,根据唯一性定理我们就能够肯 定地说,它就是该问题中旳唯一正确旳解.
静电场微分方程及唯一性定理

2 0
泊松方程和拉普拉斯方程统称为微分方程。 二、泊松方程与拉普拉斯方程适用条件 只适用于各向同性、线性的均匀媒质。(?)
§2.8.2
唯一性定理(Uniquness Theorem)
一、定理内容
在静电场中,满足给定边界条件的微分方程(泊松方程或
拉普拉斯方程)的解是唯一的,称之为静电场的唯一性定理。
2 2 2 式中: ( ex ey ez ) ( ex ey ez ) 2 2 2 2 x y z x y z x y z
2
泊松方程(针对场源点)
拉普拉斯方程(针对场点,ρ=0)
《电磁场理论》
主讲教师:李志刚 辽宁科技大学电信学院通信系 2012年05月
§2.8 静电场边值问题 唯一性定理
§2.8.1 泊松方程与拉普拉斯方程 一、静电场微分方程
D
E E E
E
E 0
常数
二、物理角度理解
场源相同、场分布相同,则场一定相同。
三、数学角度理解
方程相同、边界条件相同,则解一定相同。
四、唯一性定理的作用
1、确定何为相同场的判定条件;
2、可以采用等效方法进行问题的求解,只要保证满足唯一
性定理的条件,则解法不同,但解却一
ZJH_2-2 唯一性定理_p18

∂n
S
V
Qi ∂n
∫
证明( 证明(反证法) 反证法):设有两个不同的电势均满足泊松方程 令 Φ = ϕ '−ϕ " ∂ϕ ' Qi − dS = Laplace Eq. ∇2Φ = 0 对每个导体 ∫S ∂Φ i ∂n −∫ dS = 0 ε P276 (I.7) Si ∂n ∂ϕ " Qi 面积分 −∫ dS = →体积分 对于扣除导体的空间体积,考虑积分 Si ∂n ε 对于扣除导体的空间体积V内给定自由电荷分布 ρ( ρ ϕ 满足∇2ϕ = − V ρ( x) ε ∂ϕ 在V边界S上给定 电势 ϕ S 或电势的法向导数 ,
则V内的电场(静电场)唯一地确定。 唯一地确定。 (1) 在区域V中每个均匀的子区域Vi内满 ρ 2 足泊松方程 ∇ϕ = (i = 1,2,......)
§2-2-1 均匀单一介质情形的唯一性定理 x) 对均匀单一介质, 区域V内给定自由电荷分布 ρ( ϕS ρ 2 ϕ 满足∇ ϕ = − V ρ( x) ε ∂ϕ ∂ϕ 在V边界S上给定 电势 ϕ S 或电势的法向导数 , ∂n S 则V内的电场(静电场)唯一地确定。 唯一地确定。 ∂n S 或: 若给定求解区域V内自由电荷ρ 内自由电荷ρ分布和介质的性质ε 分布和介质的性质ε, 以及在边界面上的 (1)电势值( 电势值(第一类边界条件), 第一类边界条件), 或(2)电势法向导数( 电势法向导数(第二类边界条件), 第二类边界条件), 或(3)一部分的电势值, 一部分的电势值,其余部分的电势法向导数值 (第三类边界条件), 第三类边界条件), (则在区域V内Possion方程( 方程(或Laplace方程) 方程)的解是惟一的。 的解是惟一的。
电动力学2-2 唯一性定理

E1t = E2t A E1 = 3 r r
D2n = D n = 0 1
∫ D⋅ dS = ∫ ε E ⋅ dS + ∫
S1 1 1
S2
ε2 E2 ⋅ dS = Q
将电场值代入得 2π (ε1 +ε2 ) A= Q Q A= 解出 2π (ε1 +ε2 )
Qr 则 E1 = (左半部 左半部) 左半部 3 2π (ε1 + ε2 )r
2
在两区域Vi和Vj的分界面上满足边值关系 在两区域
∂ϕ ∂ϕ ϕi = ϕ j, εi = ε j ∂n i ∂n j
除此之外,要完全确定 内的电场 还必须给出V 内的电场, 除此之外,要完全确定V内的电场,还必须给出 内的边界S上的一些条件。 内的边界 上的一些条件。下面提出的唯一性定 上的一些条件 理具体指出所需给定的边界条件。 理具体指出所需给定的边界条件。
Qr E2 = 右半部) 右半部 3 (右半部 2π (ε1 + ε2 )r
此解满足唯一性定理的所有条件, 此解满足唯一性定理的所有条件,因此是唯一正 确的解。 确的解。 虽然E仍保持球对称性,但是 和导体面上的电荷 虽然 仍保持球对称性,但是D和导体面上的电荷 仍保持球对称性 面密度σ不具有球对称性。设内导体半径为 , 面密度 不具有球对称性。设内导体半径为a,则 不具有球对称性 球面上的电荷面密度为
§2.2 唯一性定理
一、唯一性定理的重要意义
1. 给出了确定静电场的条件,这是解决实际问题 给出了确定静电场的条件, 的依据。 的依据。 2. 在有解的情况下 , 解是唯一的 。 因此 , 在实 在有解的情况下, 解是唯一的。 因此, 际问题中,可以根据给定的条件作一定的分析, 际问题中,可以根据给定的条件作一定的分析, 提出尝试解, 提出尝试解,只要它满足唯一性定理所要求的条 件,它就是唯一正确的解。 它就是唯一正确的解。
2.2唯一性定理

结束
第二章∶静电场
因此
i i i
i
dsi ds n
Si
ds S
S
这里S为区域(总)边界, 代 表总边界处介质的介电常数,
S [ ] ds = V ( )d = ( ) ( ) d V
i
i
2
i
2 [ ] ds ( ) ( ) d
Si
Vi
令 i i , = i 注意到 2 i 0,有
i i
(应为一确定值)
故可从积分号内提出来,于是
V
( ) d
2
Si
ds n
现在分析:
Si
ds ? n
结束
第二章∶静电场
因为Si表示电场中第i个导体的表面。静电平衡时, 导体外紧靠导体表面处的场强方向与表面垂直,场 强的大小与表面对应点的面电荷密度成正比,即
2
S 0 or 0 n S
2
V ( ) d Si n ds
结束
第二章∶静电场
这里导体表面Si处的电势并没有给定,但由于导体
在静电平衡时为一等势体。虽然Si与Si不一
定相等,但对同一导体而言,两者在导体表面各
处的差值
S S i
Si
i i i dsi = i ( i )2 d i
Vi
考虑左边的面积分,在两个均匀区域Vi 和Vj 的界 面上, 由于 j i i j , i j n n 结束
关于静电场中唯一性定理的证明

关于静电场中唯一性定理的证明
静电场中唯一性定理:满足静电场的**Maxwell方程组的唯一解,取决于指定的边界条件而不受初始条件的约束。
为了证明该定理,我们首先考虑Maxwell方程组:
$\nabla\cdot\vec{E} = \frac{\rho}{\varepsilon_0}$
可以看出,这套方程是由边界条件决定的,其解也是由边界条件决定的。
为证明唯一性定理,我们使用变分法从而得出以下**Euler-Lagrange方程组:
$\frac{\partial L}{\partial \vec{E}}-\frac{\partial}{\partial
\vec{x}}\frac{\partial L}{\partial(\frac{\partial\vec{E}}{\partial
x})}+\frac{\partial}{\partial t}\frac{\partial L}{\partial\frac{\partial
\vec{E}}{\partial t}}=0$
其中,$L$表示Lagrange函数,它是由Maxwell方程组构成的。
由此,我们可以得出雅可比方程:
这组方程有两个基本性质,一是称为“唯一性原理”,一是称为“不变性定理”。
不变性定理:对于给定的满足Maxwell方程组的特定边界条件,解不会随着时间变化而变化。
这两个定理说明,解是唯一的,而且不受初始条件的限制,而只受边界条件的约束。
因此,以上证明了静电场中唯一性定理。
静电场边值问题的唯一性定理共21页文档

35、不要以为自己成功一次就可以了 ,也不 要以为 过去的 光荣可 以被永 远肯定 。
静电场边值问题的唯一性定 理
31、别人笑我太疯癫,我笑他人看不 穿。(名 言网) 32、我不想听失意者的哭泣,抱怨者 的牢骚 ,这是 羊群中 的瘟疫 ,我不 能被它 传染。 我要尽 避免以 失败收 常在别 人停滞 不前时 ,我继 续拼搏 。
45、自己的饭量自己知道。——苏联
41、学问是异常珍贵的东西,从任何源泉吸 收都不可耻。——阿卜·日·法拉兹
42、只有在人群中间,才能认识自 己。——德国
43、重复别人所说的话,只需要教育; 而要挑战别人所说的话,则需要头脑。—— 玛丽·佩蒂博恩·普尔
44、卓越的人一大优点是:在不利与艰 难的遭遇里百折不饶。——贝多芬
静电场电位边值问题唯一性定理的补充与完整证明

静电场电位边值问题唯一性定理的补充与完整证明陈文卿;闫述【摘要】The electrostatic boundary value problem and the uniqueness of solutions are sup-plemented and proved in this paper.At first,the region condition and the convergence bound-ary are distinguished from the usual mixed singularity.The form of Robin Problem in electro-static field boundary value problem is confirmed.The convergence condition and the infinite boundary condition are added to the uniqueness theorem of solutions.These boundary condi-tions are re-classified according to the form of mathematical expressions.Then in the proof of the uniqueness of the potential solutions under boundary conditions,infinite boundary condi-tions and convergence conditions,the problem of the coefficient of the third kind of boundary condition and the applicative boundary value problem with infinite space are solved.We also demonstrate the uniqueness of potential solutions for Dirichlet and Robin Problem and con-stant differences in the potential of Neumann Problem.Finally,the application of region,in-finity and convergence boundary conditions in problems solving is illustrated by an example.The supplemented theorem can be better used as the basis for solving problems and follow-up learning.%本文对静电场电位边值问题与解的唯一性定理作了补充与完整的证明.首先将区域边界与衔接边界从通常的混称中区分开来,确认了静电场边值问题中第三类边界条件应有的形式,在解的唯一性定理中增加了衔接条件和无限远边界条件,并根据数学表达式的形式重新归类.然后在区域边界条件、无限远边界条件和衔接条件下电位解的唯一性的证明中,讨论了第一、第三类边值问题电位解的唯一性与全二类边界条件下电位存在常数差的问题,解除了第三类边界条件系数为正的限制,说明了整个求解空间为无限大时适用的边值问题.最后通过例题说明了区域、无限远和衔接3种边界条件在解题中的应用.补充后的定理可以更好地作为解题和后续学习的依据和基础.【期刊名称】《物理与工程》【年(卷),期】2017(027)006【总页数】6页(P54-59)【关键词】电位的边值问题;区域边界条件;衔接条件;唯一性定理;证明【作者】陈文卿;闫述【作者单位】江苏大学计算机科学与通信工程学院,江苏镇江 212013;江苏大学计算机科学与通信工程学院,江苏镇江 212013【正文语种】中文电位的边值问题与解的唯一性是通信和电子信息类相关专业本科阶段电磁场与电磁波和电动力学课程中静电场部分的重要内容,也是求解其他边值问题的基础。
第二章第二节 唯一性定理

ϕi ' = ϕ j '
∂ϕ j ' ∂ϕ i ' εi =εj ∂n ∂n
ϕi ' ' = ϕ j ' '
∂ϕ j ' ' ∂ϕ i ' ' εi =εj ∂n ∂n
Vj
因此,在介质分界面上, 因此,在介质分界面上,ϕ也满足
Vi
ϕi = ϕ j
∂ϕ j ∂ϕ i εi =εj ∂n ∂n
——(2.5)
运用唯一性定理讨论几个问题
例一: 例一:有一个中性的导体球壳 A,在此球壳内放 置一带电体 M,其荷电为 Q。证明: 1) 球壳外的电场只与 Q有关, 与 M在球壳内的位置无关; 2) 球壳 A的外表面上的电荷为 均匀分布,与 M在球壳内的 位置无关。
S
M
证明: 证明: 所研究的区域为球壳外的区域, 其界面为 S∞ 和 S 。 边界 S∞ 上的电势为零; 而对于界面S,由于感应使得 S的内表面的电量为 -Q,则界面 S上的总电量为 +Q,这一结论不 论M在球壳内何处,只要在球壳 内即成立。
∫
Si
ϕ∇ϕ ⋅ dS = −ϕ i ∫ ∇ϕ ⋅ dS
Si
V V’
=0
而对于外边界面 S,根据(2.13) 外边界面 可知,
i
Si
∫ ϕ ∇ ϕ ⋅ dS = 0
S
n S
对于区域 V 的外表面 S
ϕ S = 0 或者 ∂ϕ ∂n S = 0 ——(2.13)
V
因此,对 V’ 的整个界面
V’
∫ ϕ ∇ ϕ ⋅ dS = 0
2 i Vi i
Vj
但是被积函数始终满足
Vi
静电场唯一性定理

王向斌 静电场唯一性定理的部分内容表述
若真空区域所有边界面的条件确定了,则该真空区域的静电场 就唯一确定了. 根据此定理,不论真空区域以外(含边界)的电荷分布如何变化, 只要边界条件维持不变,则真空区域电场维持不变. (但是区域 以外的电场可能会发生变化.) 换言之,不论真空区域以外的实 际点荷分布如何,我们可以在真空区域之外构造一种简单的电 荷分布,只要它能够满足给定的真空区域边界面条件,我们就可 以按这种人为构造的电荷分布计算真空区域内的电场. (但不能 用此法计算真空区域以外的电场.) 根据此定理,只要找到一个电势函数, 能满足区域真空条件和 边界条件的要求,则真空区域内的电场可由该函数算出. (真空区域以外的电场不可以.)
思考题: 上述封闭面S在引理和定理中,是否必需是导体面? 还是任何满足面上电势要求的数学面都可以? 思考题: 在哪里用到或者隐含用到了势函数满足区域真空条件?
应用
静电屏蔽,电像法, 其他计算问题 思考题: 电像法中,像电荷为什么必需在真空区域以外? 思考题: 课本的电像法例题中,利用了唯一性定理.究竟是怎样与 唯一性定理的边界条件一一对应的? 即,接地的无限大金属板以及 题中的点电荷应该理解成唯一性定理的哪一个边界面?
引理2: 引理1中,若封闭面S是带电量为0的等势面,结论依然成立.
唯一性定理的部分内容的证明
条件: 静电场情况; 封闭面S, 该面电势函数确定;S面内部最多有3类区域: 真空区域, 电势确定的的导体区域,和带电量确定的导体区域.
依据唯一性定理, 上述真空区域的电场唯一确定. 思路: 真空区域若有两个势函数,函数1和函数2都满足边界条件 和区域真空条件, 把这两个势函数之差看成第三个势函数,由于 每个势函数边界条件都一样, 第三个势函数的边界条件必然是 引理1中的边界条件,因而第三个势函数在真空区域是等势区域, 此即说明函数1和函数2在真空区域最多只相差一个常数,因此给 出相同的电场. 思考题: 为什么两个电势函数之差这样一个数学函数一定可以 看成一个电势函数?
静电场边值问题的唯一性定理

U Qk e dS 0 En dS 0 dS n Sk Sk Sk
U 0 dS 0 U U I U II 常量 EI EII n Sk
说明场分布是唯一的
解释静电屏蔽
唯一性定理表明:一旦找到某种电荷分布,既不 违背导体平衡特性,又是物理实在,则这种电荷 分布就是唯一可能的分布。
推广:若完全由导体所包围的空间里各导体
的电势都相等(设为U0),则空间电势等于 常量U0
引理三:若所有导体都不带电, 则各导体的电势都相等
证明(反证)
若不相等,必有一个最高, 如图设U1>U2、U3,——导 体1是电场线的起点——其 表面只有正电荷——导体1 上的总电量不为0——与前 提矛盾
引理二
( +)引理三可推论:所有导体都不带电的 情况下空间各处的电势也和导体一样,等于同一常 量
叠加原理
在给定各带电导体的几何形状、相对位置后,赋予 两组边界条件:
1:给定每个导体的电势UⅠk(或总电量QⅠk) 2:给定每个导体的电势UⅡk(或总电量QⅡk) 设UⅠ、 UⅡ满足上述两条件,则它们的线性组合 U=a UⅠ+b UⅡ必满足条件3: 3:给定每个导体的电势Uk=a UⅠk+b UⅡ k (或总电量Qk= QⅠk a k+b QⅡ k) 特例 : 取UⅠk= UⅡ k,则U=UⅠ-UⅡ (a=1, b=-1) 对应 的边界条件为,每个导体的电势为0
唯一性定理
给定每个导体电势的情形
设对应同一组边值 U k (k 1,2)
有两种恒定的电势分布 U I 和U II
相当于所有导 体上电势为0时 的恒定电势分 布
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
3. Mixed boundary-value condition: The physical quantities on some boundaries are given, and the normal derivatives of the physical quantities are specified on the remaining boundaries.
For any mathematical physics equation, the existence, the stability, and the uniqueness of the solutions need to be investigated.
Chapter 3 Boundary-Value Problems in Electrostatics
Differential Equations for Electric Potential Method of Images
Method of Separation of Variables
1.Differential Equations for Electric Potential 2.Method of Image 3.Method of Separation of Variables in Rectangular Coordinates 4.Method of Separation of Variables in Cylindrical Coordinates 5.Method of Separation of Variables in Spherical Coordinates
in V produces the electric potential given by
(r)1 4πV|(r ) r r
|dV
(rco)nfined to
which is just the solution for Poisson’s Equation in free space.
Applying Green’s function G(r, r) gives the general solution of
G0 (r,
r)
1 4π | r r |
In the source-free region, the volume integral in the above equation will be zero. Therefore, the second surface integral is considered to be the solution of Poisson’s equation in source-free region, or the integral solution of Laplace’s equation in terms of Green’s function.
An equation in mathematical physics is to describe the changes of physical quantities with respect to space and time. For the specified region and moment, the solution of an equation depends on the initial condition and the boundary condition, respectively, and both are also called the solving condition.
equation gives
E 2
In a linear, homogeneous, and isotropic medium, the divergence of the electric field intensity E is
E
The differential equation for the electric potential is
Poission’s equation
(r)
V G(r,
r) ( r) dV
S [G(r, r)(r) (r)G(r, r)] dS
For infinite free space, the surface integral in the above equation will
become zero, and Green’s function becomes
Usually the boundary conditions are classified into three types:
1. Dirichet boundary condition: The physical quantities on the boundaries are specified.
1. Differential Equations for Electric Potential
The relationship between the electric potential and the electric field intensity E is
E
Taking the divergence operation for both sides of the above
2
which is called Poisson’s equation.
In a source-free region, and the above equation becomes
2 0
which is called Laplace’s equation.
The solution of Poisson’s InEinqfuinatitioenfr. ee space, the electric charge density
