数学专业英语课文翻译2-9,2-10

数学专业英语2-10翻译

Although dependence and independence are properties of sets of elements, we also apply these terms to the elements themselves. For example, the elements in an independent set are called independent elements.虽然相关和无关是元素集的属性，我们也适用于这些元素本身。

例如，在一个独立设定的元素被称为独立元素。

If s is finite set, the foregoing definition agrees with that given in Chapter 8 for the space n V . However, the present definition is not restricted to finite sets.如果S 是有限集，同意上述定义与第8章中给出的空间n V ，然而，目前的定义不局限于有限集。

If a subset T of a set S is dependent, then S itself is dependent. This is logically equivalent to the statement that every subset of an independent set is independent.如果集合S 的子集T 是相关的，然后S 本身是相关的，这在逻辑上相当于每一个独立设置的子集是独立的语句。

If one element in S is a scalar multiple of another, then S is dependent. 如果S 中的一个元素是另一个集中的多个标量的，则S 是相关的。

If S ∈0,then S is dependent. 若S ∈0，则 S 是相关的。

数学专业英语第二版-课文翻译-converted

2.4 整数、有理数与实数4－A Integers and rational numbersThere exist certain subsets of R which are distinguished because they have special properties not shared by all real numbers. In this section we shall discuss such subsets, the integers and the rational numbers.有一些R 的子集很著名，因为他们具有实数所不具备的特殊性质。

在本节我们将讨论这样的子集，整数集和有理数集。

To introduce the positive integers we begin with the number 1, whose existence is guaranteed by Axiom 4. The number 1+1 is denoted by 2, the number 2+1 by 3, and so on. The numbers 1,2,3,…, obtained in this way by repeated addition of 1 are all positive, and they are called the positive integers.我们从数字 1 开始介绍正整数，公理 4 保证了 1 的存在性。

1+1 用2 表示，2+1 用3 表示，以此类推，由 1 重复累加的方式得到的数字 1,2,3，…都是正的，它们被叫做正整数。

Strictly speaking, this description of the positive integers is not entirely complete because we have not explained in detail what we mean by the expressions “and so on”, or “repeated addition of 1”.严格地说，这种关于正整数的描述是不完整的，因为我们没有详细解释“等等”或者“1的重复累加”的含义。

高等数学专业名词中英文对照(全面)

微积分英文词汇,高数名词中英文对照,高等数学术语英语翻译一览关键词：微积分英文,高等数学英文翻译,高数英语词汇来源：上海外教网 | 发布日期：2008-05-16 17:12V、X、Z:Value of function ：函数值Variable ：变数Vector ：向量Velocity ：速度Vertical asymptote ：垂直渐近线Volume ：体积X-axis ：x轴x-coordinate ：x坐标x-intercept ：x截距Zero vector ：函数的零点Zeros of a polynomial ：多项式的零点T:Tangent function ：正切函数Tangent line ：切线Tangent plane ：切平面Tangent vector ：切向量Total differential ：全微分Trigonometric function ：三角函数Trigonometric integrals ：三角积分Trigonometric substitutions ：三角代换法Tripe integrals ：三重积分S:Saddle point ：鞍点Scalar ：纯量Secant line ：割线Second derivative ：二阶导数Second Derivative Test ：二阶导数试验法Second partial derivative ：二阶偏导数Sector ：扇形Sequence ：数列Series ：级数Set ：集合Shell method ：剥壳法Sine function ：正弦函数Singularity ：奇点Slant asymptote ：斜渐近线Slope ：斜率Slope-intercept equation of a line ：直线的斜截式Smooth curve ：平滑曲线Smooth surface ：平滑曲面Solid of revolution ：旋转体Space ：空间Speed ：速率Spherical coordinates ：球面坐标Squeeze Theorem ：夹挤定理Step function ：阶梯函数Strictly decreasing ：严格递减Strictly increasing ：严格递增Sum ：和Surface ：曲面Surface integral ：面积分Surface of revolution ：旋转曲面Symmetry ：对称R:Radius of convergence ：收敛半径Range of a function ：函数的值域Rate of change ：变化率Rational function ：有理函数Rationalizing substitution ：有理代换法Rational number ：有理数Real number ：实数Rectangular coordinates ：直角坐标Rectangular coordinate system ：直角坐标系Relative maximum and minimum ：相对极大值与极小值Revenue function ：收入函数Revolution , solid of ：旋转体Revolution , surface of ：旋转曲面Riemann Sum ：黎曼和Riemannian geometry ：黎曼几何Right-hand derivative ：右导数Right-hand limit ：右极限Root ：根P、Q:Parabola ：拋物线Parabolic cylinder ：抛物柱面Paraboloid ：抛物面Parallelepiped ：平行六面体Parallel lines ：并行线Parameter ：参数Partial derivative ：偏导数Partial differential equation ：偏微分方程Partial fractions ：部分分式Partial integration ：部分积分Partiton ：分割Period ：周期Periodic function ：周期函数Perpendicular lines ：垂直线Piecewise defined function ：分段定义函数Plane ：平面Point of inflection ：反曲点Polar axis ：极轴Polar coordinate ：极坐标Polar equation ：极方程式Pole ：极点Polynomial ：多项式Positive angle ：正角Point-slope form ：点斜式Power function ：幂函数Product ：积Quadrant ：象限Quotient Law of limit ：极限的商定律Quotient Rule ：商定律M、N、O:Maximum and minimum values ：极大与极小值Mean Value Theorem ：均值定理Multiple integrals ：重积分Multiplier ：乘子Natural exponential function ：自然指数函数Natural logarithm function ：自然对数函数Natural number ：自然数Normal line ：法线Normal vector ：法向量Number ：数Octant ：卦限Odd function ：奇函数One-sided limit ：单边极限Open interval ：开区间Optimization problems ：最佳化问题Order ：阶Ordinary differential equation ：常微分方程Origin ：原点Orthogonal ：正交的L:Laplace transform ：Leplace 变换Law of Cosines ：余弦定理Least upper bound ：最小上界Left-hand derivative ：左导数Left-hand limit ：左极限Lemniscate ：双钮线Length ：长度Level curve ：等高线L'Hospital's rule ：洛必达法则Limacon ：蚶线Limit ：极限Linear approximation：线性近似Linear equation ：线性方程式Linear function ：线性函数Linearity ：线性Linearization ：线性化Line in the plane ：平面上之直线Line in space ：空间之直线Lobachevski geometry ：罗巴切夫斯基几何Local extremum ：局部极值Local maximum and minimum ：局部极大值与极小值Logarithm ：对数Logarithmic function ：对数函数I:Implicit differentiation ：隐求导法Implicit function ：隐函数Improper integral ：瑕积分Increasing/Decreasing Test ：递增或递减试验法Increment ：增量Increasing Function ：增函数Indefinite integral ：不定积分Independent variable ：自变数Indeterminate from ：不定型Inequality ：不等式Infinite point ：无穷极限Infinite series ：无穷级数Inflection point ：反曲点Instantaneous velocity ：瞬时速度Integer ：整数Integral ：积分Integrand ：被积分式Integration ：积分Integration by part ：分部积分法Intercepts ：截距Intermediate value of Theorem ：中间值定理Interval ：区间Inverse function ：反函数Inverse trigonometric function ：反三角函数Iterated integral ：逐次积分H:Higher mathematics 高等数学/高数E、F、G、H:Ellipse ：椭圆Ellipsoid ：椭圆体Epicycloid ：外摆线Equation ：方程式Even function ：偶函数Expected Valued ：期望值Exponential Function ：指数函数Exponents , laws of ：指数率Extreme value ：极值Extreme Value Theorem ：极值定理Factorial ：阶乘First Derivative Test ：一阶导数试验法First octant ：第一卦限Focus ：焦点Fractions ：分式Function ：函数Fundamental Theorem of Calculus ：微积分基本定理Geometric series ：几何级数Gradient ：梯度Graph ：图形Green Formula ：格林公式Half-angle formulas ：半角公式Harmonic series ：调和级数Helix ：螺旋线Higher Derivative ：高阶导数Horizontal asymptote ：水平渐近线Horizontal line ：水平线Hyperbola ：双曲线Hyper boloid ：双曲面D:Decreasing function ：递减函数Decreasing sequence ：递减数列Definite integral ：定积分Degree of a polynomial ：多项式之次数Density ：密度Derivative ：导数of a composite function ：复合函数之导数of a constant function ：常数函数之导数directional ：方向导数domain of ：导数之定义域of exponential function ：指数函数之导数higher ：高阶导数partial ：偏导数of a power function ：幂函数之导数of a power series ：羃级数之导数of a product ：积之导数of a quotient ：商之导数as a rate of change ：导数当作变率right-hand ：右导数second ：二阶导数as the slope of a tangent ：导数看成切线之斜率Determinant ：行列式Differentiable function ：可导函数Differential ：微分Differential equation ：微分方程partial ：偏微分方程Differentiation ：求导法implicit ：隐求导法partial ：偏微分法term by term ：逐项求导法Directional derivatives ：方向导数Discontinuity ：不连续性Disk method ：圆盘法Distance ：距离Divergence ：发散Domain ：定义域Dot product ：点积Double integral ：二重积分change of variable in ：二重积分之变数变换in polar coordinates ：极坐标二重积分C:Calculus ：微积分differential ：微分学integral ：积分学Cartesian coordinates ：笛卡儿坐标一般指直角坐标Cartesian coordinates system ：笛卡儿坐标系Cauch’s Mean Value Theor em ：柯西均值定理Chain Rule ：连锁律Change of variables ：变数变换Circle ：圆Circular cylinder ：圆柱Closed interval ：封闭区间Coefficient ：系数Composition of function ：函数之合成Compound interest ：复利Concavity ：凹性Conchoid ：蚌线Cone ：圆锥Constant function ：常数函数Constant of integration ：积分常数Continuity ：连续性at a point ：在一点处之连续性of a function ：函数之连续性on an interval ：在区间之连续性from the left ：左连续from the right ：右连续Continuous function ：连续函数Convergence ：收敛interval of ：收敛区间radius of ：收敛半径Convergent sequence ：收敛数列series ：收敛级数Coordinate：s：坐标Cartesian ：笛卡儿坐标cylindrical ：柱面坐标polar ：极坐标rectangular ：直角坐标spherical ：球面坐标Coordinate axes ：坐标轴Coordinate planes ：坐标平面Cosine function ：余弦函数Critical point ：临界点Cubic function ：三次函数Curve ：曲线Cylinder：圆柱Cylindrical Coordinates ：圆柱坐标A、B:Absolute convergence ：绝对收敛Absolute extreme values ：绝对极值Absolute maximum and minimum ：绝对极大与极小Absolute value ：绝对值Absolute value function ：绝对值函数Acceleration ：加速度Antiderivative ：反导数Approximate integration ：近似积分Approximation ：逼近法by differentials ：用微分逼近linear ：线性逼近法by Simpson’s Rule ：Simpson法则逼近法by the Trapezoidal Rule ：梯形法则逼近法Arbitrary constant ：任意常数Arc length ：弧长Area ：面积under a curve ：曲线下方之面积between curves ：曲线间之面积in polar coordinates ：极坐标表示之面积of a sector of a circle ：扇形之面积of a surface of a revolution ：旋转曲面之面积Asymptote ：渐近线horizontal ：水平渐近线slant ：斜渐近线vertical ：垂直渐近线Average speed ：平均速率Average velocity ：平均速度Axes, coordinate ：坐标轴Axes of ellipse ：椭圆之轴Binomial series ：二项级数微积分词汇第一章函数与极限Chapter1 Function and Limit集合 set元素 element子集 subset空集 empty set并集 union交集 intersection差集 difference of set基本集 basic set补集 complement set直积 direct product笛卡儿积 Cartesian product开区间 open interval闭区间 closed interval半开区间 half open interval有限区间 finite interval区间的长度 length of an interval无限区间 infinite interval领域 neighborhood领域的中心 centre of a neighborhood 领域的半径 radius of a neighborhood 左领域 left neighborhood右领域 right neighborhood映射 mappingX到Y的映射 mapping of X ontoY满射 surjection单射 injection一一映射 one-to-one mapping双射 bijection算子 operator变化 transformation函数 function逆映射 inverse mapping复合映射 composite mapping自变量 independent variable因变量 dependent variable定义域 domain函数值 value of function函数关系 function relation值域 range自然定义域 natural domain单值函数 single valued function多值函数 multiple valued function单值分支 one-valued branch函数图形 graph of a function绝对值函数 absolute value符号函数 sigh function整数部分 integral part阶梯曲线 step curve当且仅当 if and only if(iff)分段函数 piecewise function上界 upper bound下界 lower bound有界 boundedness无界 unbounded函数的单调性 monotonicity of a function单调增加的 increasing单调减少的 decreasing单调函数 monotone function函数的奇偶性 parity(odevity) of a function 对称 symmetry偶函数 even function奇函数 odd function函数的周期性 periodicity of a function周期 period反函数 inverse function直接函数 direct function复合函数 composite function中间变量 intermediate variable函数的运算 operation of function基本初等函数 basic elementary function初等函数 elementary function幂函数 power function指数函数 exponential function对数函数 logarithmic function三角函数 trigonometric function反三角函数 inverse trigonometric function 常数函数 constant function双曲函数 hyperbolic function双曲正弦 hyperbolic sine双曲余弦 hyperbolic cosine双曲正切 hyperbolic tangent反双曲正弦 inverse hyperbolic sine反双曲余弦 inverse hyperbolic cosine反双曲正切 inverse hyperbolic tangent极限 limit数列 sequence of number收敛 convergence收敛于 a converge to a发散 divergent极限的唯一性 uniqueness of limits收敛数列的有界性 boundedness of a convergent sequence子列 subsequence函数的极限 limits of functions函数当x趋于x0时的极限 limit of functions as x approaches x0左极限 left limit右极限 right limit单侧极限 one-sided limits水平渐近线 horizontal asymptote无穷小 infinitesimal无穷大 infinity铅直渐近线 vertical asymptote夹逼准则 squeeze rule单调数列 monotonic sequence高阶无穷小 infinitesimal of higher order低阶无穷小 infinitesimal of lower order同阶无穷小 infinitesimal of the same order作者：新少年特工 2007-10-8 18:37 回复此发言--------------------------------------------------------------------------------2 高等数学-翻译等阶无穷小 equivalent infinitesimal函数的连续性 continuity of a function增量 increment函数在x0连续 the function is continuous at x0左连续 left continuous右连续 right continuous区间上的连续函数 continuous function函数在该区间上连续 function is continuous on an interval不连续点 discontinuity point第一类间断点 discontinuity point of the first kind第二类间断点 discontinuity point of the second kind初等函数的连续性 continuity of the elementary functions定义区间 defined interval最大值 global maximum value (absolute maximum)最小值 global minimum value (absolute minimum)零点定理 the zero point theorem介值定理 intermediate value theorem第二章导数与微分Chapter2 Derivative and Differential速度 velocity匀速运动 uniform motion平均速度 average velocity瞬时速度 instantaneous velocity圆的切线 tangent line of a circle切线 tangent line切线的斜率 slope of the tangent line位置函数 position function导数 derivative可导 derivable函数的变化率问题 problem of the change rate of a function导函数 derived function左导数 left-hand derivative右导数 right-hand derivative单侧导数 one-sided derivatives在闭区间【a,b】上可导 is derivable on the closed interval [a,b] 切线方程 tangent equation角速度 angular velocity成本函数 cost function边际成本 marginal cost链式法则 chain rule隐函数 implicit function显函数 explicit function二阶函数 second derivative三阶导数 third derivative高阶导数 nth derivative莱布尼茨公式 Leibniz formula对数求导法 log- derivative参数方程 parametric equation相关变化率 correlative change rata微分 differential可微的 differentiable函数的微分 differential of function自变量的微分 differential of independent variable微商 differential quotient间接测量误差 indirect measurement error绝对误差 absolute error相对误差 relative error第三章微分中值定理与导数的应用Chapter3 MeanValue Theorem of Differentials and the Application of Derivatives罗马定理Rolle’s theorem费马引理Fermat’s lemma拉格朗日中值定理Lagrange’s mean value theorem驻点 stationary point稳定点 stable point临界点 critical point辅助函数 auxiliary function拉格朗日中值公式Lagrange’s mean value formula柯西中值定理Cauchy’s mean value theorem洛必达法则L’Hospital’s Rule0/0型不定式 indeterminate form of type 0/0不定式 indeterminate form泰勒中值定理Taylor’s mean value theorem泰勒公式 Taylor formula余项 remainder term拉格朗日余项 Lagrange remainder term麦克劳林公式Maclaurin’s formula佩亚诺公式 Peano remainder term凹凸性 concavity凹向上的 concave upward, cancave up凹向下的，向上凸的concave downward’ concave down拐点 inflection point函数的极值 extremum of function极大值 local(relative) maximum最大值 global(absolute) mximum极小值 local(relative) minimum最小值 global(absolute) minimum目标函数 objective function曲率 curvature弧微分 arc differential平均曲率 average curvature曲率园 circle of curvature曲率中心 center of curvature曲率半径 radius of curvature渐屈线 evolute渐伸线 involute根的隔离 isolation of root隔离区间 isolation interval切线法 tangent line method第四章不定积分Chapter4 Indefinite Integrals原函数 primitive function(antiderivative)积分号 sign of integration被积函数 integrand积分变量 integral variable积分曲线 integral curve积分表 table of integrals换元积分法 integration by substitution分部积分法 integration by parts分部积分公式 formula of integration by parts有理函数 rational function真分式 proper fraction假分式 improper fraction第五章定积分Chapter5 Definite Integrals曲边梯形 trapezoid with曲边 curve edge窄矩形 narrow rectangle曲边梯形的面积 area of trapezoid with curved edge积分下限 lower limit of integral积分上限 upper limit of integral积分区间 integral interval分割 partition积分和 integral sum可积 integrable矩形法 rectangle method积分中值定理 mean value theorem of integrals函数在区间上的平均值 average value of a function on an integvals 牛顿－莱布尼茨公式 Newton-Leibniz formula微积分基本公式 fundamental formula of calculus换元公式 formula for integration by substitution递推公式 recurrence formula反常积分 improper integral反常积分发散 the improper integral is divergent反常积分收敛 the improper integral is convergent无穷限的反常积分 improper integral on an infinite interval无界函数的反常积分 improper integral of unbounded functions绝对收敛 absolutely convergent第六章定积分的应用Chapter6 Applications of the Definite Integrals元素法 the element method面积元素 element of area平面图形的面积 area of a luane figure直角坐标又称“笛卡儿坐标(Cartesian coordinates)”极坐标 polar coordinates抛物线 parabola椭圆 ellipse旋转体的面积 volume of a solid of rotation旋转椭球体 ellipsoid of revolution, ellipsoid of rotation 曲线的弧长 arc length of acurve可求长的 rectifiable光滑 smooth功 work水压力 water pressure引力 gravitation变力 variable force第七章空间解析几何与向量代数Chapter7 Space Analytic Geometry and Vector Algebra向量 vector自由向量 free vector单位向量 unit vector零向量 zero vector相等 equal平行 parallel向量的线性运算 linear poeration of vector三角法则 triangle rule平行四边形法则 parallelogram rule交换律 commutative law结合律 associative law负向量 negative vector差 difference分配律 distributive law空间直角坐标系 space rectangular coordinates坐标面 coordinate plane卦限 octant向量的模 modulus of vector向量a与b的夹角 angle between vector a and b方向余弦 direction cosine方向角 direction angle向量在轴上的投影 projection of a vector onto an axis数量积，外积，叉积 scalar product,dot product,inner product 曲面方程 equation for a surface球面 sphere旋转曲面 surface of revolution母线 generating line轴 axis圆锥面 cone顶点 vertex旋转单叶双曲面 revolution hyperboloids of one sheet旋转双叶双曲面 revolution hyperboloids of two sheets柱面 cylindrical surface ,cylinder圆柱面 cylindrical surface准线 directrix抛物柱面 parabolic cylinder二次曲面 quadric surface椭圆锥面 dlliptic cone椭球面 ellipsoid单叶双曲面 hyperboloid of one sheet双叶双曲面 hyperboloid of two sheets旋转椭球面 ellipsoid of revolution椭圆抛物面 elliptic paraboloid旋转抛物面 paraboloid of revolution双曲抛物面 hyperbolic paraboloid马鞍面 saddle surface椭圆柱面 elliptic cylinder双曲柱面 hyperbolic cylinder抛物柱面 parabolic cylinder空间曲线 space curve空间曲线的一般方程 general form equations of a space curve 空间曲线的参数方程 parametric equations of a space curve 螺转线 spiral螺矩 pitch投影柱面 projecting cylinder投影 projection平面的点法式方程 pointnorm form eqyation of a plane法向量 normal vector平面的一般方程 general form equation of a plane两平面的夹角 angle between two planes点到平面的距离 distance from a point to a plane空间直线的一般方程 general equation of a line in space方向向量 direction vector直线的点向式方程 pointdirection form equations of a line 方向数 direction number直线的参数方程 parametric equations of a line两直线的夹角 angle between two lines垂直 perpendicular直线与平面的夹角 angle between a line and a planes平面束 pencil of planes平面束的方程 equation of a pencil of planes行列式 determinant系数行列式 coefficient determinant第八章多元函数微分法及其应用Chapter8 Differentiation of Functions of Several Variables and Its Application一元函数 function of one variable多元函数 function of several variables内点 interior point外点 exterior point边界点 frontier point,boundary point聚点 point of accumulation开集 openset闭集 closed set连通集 connected set开区域 open region闭区域 closed region有界集 bounded set无界集 unbounded setn维空间 n-dimentional space二重极限 double limit多元函数的连续性 continuity of function of seveal连续函数 continuous function不连续点 discontinuity point一致连续 uniformly continuous偏导数 partial derivative对自变量x的偏导数 partial derivative with respect to independent variable x高阶偏导数 partial derivative of higher order二阶偏导数 second order partial derivative混合偏导数 hybrid partial derivative全微分 total differential偏增量 oartial increment偏微分 partial differential全增量 total increment可微分 differentiable必要条件 necessary condition充分条件 sufficient condition叠加原理 superpostition principle全导数 total derivative中间变量 intermediate variable隐函数存在定理 theorem of the existence of implicit function曲线的切向量 tangent vector of a curve法平面 normal plane向量方程 vector equation向量值函数 vector-valued function切平面 tangent plane法线 normal line方向导数 directional derivative梯度 gradient数量场 scalar field梯度场 gradient field向量场 vector field势场 potential field引力场 gravitational field引力势 gravitational potential曲面在一点的切平面 tangent plane to a surface at a point曲线在一点的法线 normal line to a surface at a point无条件极值 unconditional extreme values条件极值 conditional extreme values拉格朗日乘数法 Lagrange multiplier method拉格朗日乘子 Lagrange multiplier经验公式 empirical formula最小二乘法 method of least squares均方误差 mean square error第九章重积分Chapter9 Multiple Integrals二重积分 double integral可加性 additivity累次积分 iterated integral体积元素 volume element三重积分 triple integral直角坐标系中的体积元素 volume element in rectangular coordinate system 柱面坐标 cylindrical coordinates柱面坐标系中的体积元素 volume element in cylindrical coordinate system 球面坐标 spherical coordinates球面坐标系中的体积元素 volume element in spherical coordinate system 反常二重积分 improper double integral曲面的面积 area of a surface质心 centre of mass静矩 static moment密度 density形心 centroid转动惯量 moment of inertia参变量 parametric variable第十章曲线积分与曲面积分Chapter10 Line(Curve)Integrals and Surface Integrals对弧长的曲线积分 line integrals with respect to arc hength第一类曲线积分 line integrals of the first type对坐标的曲线积分 line integrals with respect to x,y,and z第二类曲线积分 line integrals of the second type有向曲线弧 directed arc单连通区域 simple connected region复连通区域 complex connected region格林公式 Green formula第一类曲面积分 surface integrals of the first type对面的曲面积分 surface integrals with respect to area有向曲面 directed surface对坐标的曲面积分 surface integrals with respect to coordinate elements 第二类曲面积分 surface integrals of the second type有向曲面元 element of directed surface高斯公式 gauss formula拉普拉斯算子 Laplace operator格林第一公式Green’s first formula通量 flux散度 divergence斯托克斯公式 Stokes formula环流量 circulation旋度 rotation,curl第十一章无穷级数Chapter11 Infinite Series一般项 general term部分和 partial sum余项 remainder term等比级数 geometric series几何级数 geometric series公比 common ratio调和级数 harmonic series柯西收敛准则Cauchy convergence criteria, Cauchy criteria for convergence正项级数 series of positive terms达朗贝尔判别法D’Alembert test柯西判别法 Cauchy test交错级数 alternating series绝对收敛 absolutely convergent条件收敛 conditionally convergent柯西乘积 Cauchy product函数项级数 series of functions发散点 point of divergence收敛点 point of convergence收敛域 convergence domain和函数 sum function幂级数 power series幂级数的系数 coeffcients of power series阿贝尔定理 Abel Theorem收敛半径 radius of convergence收敛区间 interval of convergence泰勒级数 Taylor series麦克劳林级数 Maclaurin series二项展开式 binomial expansion近似计算 approximate calculation舍入误差 round-off error,rounding error欧拉公式Euler’s formula魏尔斯特拉丝判别法 Weierstrass test三角级数 trigonometric series振幅 amplitude角频率 angular frequency初相 initial phase矩形波 square wave谐波分析 harmonic analysis直流分量 direct component基波 fundamental wave二次谐波 second harmonic三角函数系 trigonometric function system傅立叶系数 Fourier coefficient傅立叶级数 Forrier series周期延拓 periodic prolongation正弦级数 sine series余弦级数 cosine series奇延拓 odd prolongation偶延拓 even prolongation傅立叶级数的复数形式 complex form of Fourier series第十二章微分方程Chapter12 Differential Equation解微分方程 solve a dirrerential equation常微分方程 ordinary differential equation偏微分方程 partial differential equation,PDE微分方程的阶 order of a differential equation微分方程的解 solution of a differential equation微分方程的通解 general solution of a differential equation初始条件 initial condition微分方程的特解 particular solution of a differential equation初值问题 initial value problem微分方程的积分曲线 integral curve of a differential equation可分离变量的微分方程 variable separable differential equation隐式解 implicit solution隐式通解 inplicit general solution衰变系数 decay coefficient衰变 decay齐次方程 homogeneous equation一阶线性方程 linear differential equation of first order非齐次 non-homogeneous齐次线性方程 homogeneous linear equation非齐次线性方程 non-homogeneous linear equation常数变易法 method of variation of constant暂态电流 transient stata current稳态电流 steady state current伯努利方程 Bernoulli equation全微分方程 total differential equation积分因子 integrating factor高阶微分方程 differential equation of higher order悬链线 catenary高阶线性微分方程 linera differential equation of higher order自由振动的微分方程 differential equation of free vibration强迫振动的微分方程 differential equation of forced oscillation串联电路的振荡方程 oscillation equation of series circuit二阶线性微分方程 second order linera differential equation线性相关 linearly dependence线性无关 linearly independce二阶常系数齐次线性微分方程 second order homogeneour linear differential equation with constant coefficient二阶变系数齐次线性微分方程 second order homogeneous linear differential equation with variable coefficient特征方程 characteristic equation无阻尼自由振动的微分方程 differential equation of free vibration with zero damping固有频率 natural frequency简谐振动 simple harmonic oscillation,simple harmonic vibration微分算子 differential operator待定系数法 method of undetermined coefficient共振现象 resonance phenomenon欧拉方程 Euler equation幂级数解法 power series solution数值解法 numerial solution勒让德方程 Legendre equation微分方程组 system of differential equations常系数线性微分方程组system of linera differential equations with constant coefficientV、X、Z:Value of function ：函数值Variable ：变数Vector ：向量Velocity ：速度Vertical asymptote ：垂直渐近线Volume ：体积X-axis ：x轴x-coordinate ：x坐标x-intercept ：x截距Zero vector ：函数的零点Zeros of a polynomial ：多项式的零点T:Tangent function ：正切函数Tangent line ：切线Tangent plane ：切平面Tangent vector ：切向量Total differential ：全微分Trigonometric function ：三角函数Trigonometric integrals ：三角积分Trigonometric substitutions ：三角代换法Tripe integrals ：三重积分S:Saddle point ：鞍点Scalar ：纯量Secant line ：割线Second derivative ：二阶导数Second Derivative Test ：二阶导数试验法Second partial derivative ：二阶偏导数Sector ：扇形Sequence ：数列Series ：级数Set ：集合Shell method ：剥壳法Sine function ：正弦函数Singularity ：奇点Slant asymptote ：斜渐近线Slope ：斜率Slope-intercept equation of a line ：直线的斜截式Smooth curve ：平滑曲线Smooth surface ：平滑曲面Solid of revolution ：旋转体Space ：空间Speed ：速率Spherical coordinates ：球面坐标Squeeze Theorem ：夹挤定理Step function ：阶梯函数Strictly decreasing ：严格递减Strictly increasing ：严格递增Sum ：和Surface ：曲面Surface integral ：面积分Surface of revolution ：旋转曲面Symmetry ：对称R:Radius of convergence ：收敛半径Range of a function ：函数的值域Rate of change ：变化率Rational function ：有理函数Rationalizing substitution ：有理代换法Rational number ：有理数Real number ：实数Rectangular coordinates ：直角坐标Rectangular coordinate system ：直角坐标系Relative maximum and minimum ：相对极大值与极小值Revenue function ：收入函数Revolution , solid of ：旋转体Revolution , surface of ：旋转曲面Riemann Sum ：黎曼和Riemannian geometry ：黎曼几何Right-hand derivative ：右导数Right-hand limit ：右极限Root ：根P、Q:Parabola ：拋物线Parabolic cylinder ：抛物柱面Paraboloid ：抛物面Parallelepiped ：平行六面体Parallel lines ：并行线Parameter ：参数Partial derivative ：偏导数Partial differential equation ：偏微分方程Partial fractions ：部分分式Partial integration ：部分积分Partiton ：分割Period ：周期Periodic function ：周期函数Perpendicular lines ：垂直线Piecewise defined function ：分段定义函数Plane ：平面Point of inflection ：反曲点Polar axis ：极轴Polar coordinate ：极坐标Polar equation ：极方程式Pole ：极点Polynomial ：多项式Positive angle ：正角Point-slope form ：点斜式Power function ：幂函数Product ：积Quadrant ：象限Quotient Law of limit ：极限的商定律Quotient Rule ：商定律M、N、O:Maximum and minimum values ：极大与极小值Mean Value Theorem ：均值定理Multiple integrals ：重积分Multiplier ：乘子Natural exponential function ：自然指数函数Natural logarithm function ：自然对数函数Natural number ：自然数Normal line ：法线Normal vector ：法向量Number ：数Octant ：卦限Odd function ：奇函数One-sided limit ：单边极限Open interval ：开区间Optimization problems ：最佳化问题Order ：阶Ordinary differential equation ：常微分方程Origin ：原点Orthogonal ：正交的L:Laplace transform ：Leplace 变换Law of Cosines ：余弦定理Least upper bound ：最小上界Left-hand derivative ：左导数Left-hand limit ：左极限Lemniscate ：双钮线Length ：长度Level curve ：等高线L'Hospital's rule ：洛必达法则Limacon ：蚶线Limit ：极限Linear approximation：线性近似Linear equation ：线性方程式Linear function ：线性函数Linearity ：线性Linearization ：线性化Line in the plane ：平面上之直线Line in space ：空间之直线Lobachevski geometry ：罗巴切夫斯基几何Local extremum ：局部极值Local maximum and minimum ：局部极大值与极小值Logarithm ：对数Logarithmic function ：对数函数I:Implicit differentiation ：隐求导法Implicit function ：隐函数Improper integral ：瑕积分Increasing/Decreasing Test ：递增或递减试验法Increment ：增量Increasing Function ：增函数Indefinite integral ：不定积分Independent variable ：自变数Indeterminate from ：不定型Inequality ：不等式Infinite point ：无穷极限Infinite series ：无穷级数Inflection point ：反曲点Instantaneous velocity ：瞬时速度Integer ：整数Integral ：积分Integrand ：被积分式Integration ：积分Integration by part ：分部积分法Intercepts ：截距Intermediate value of Theorem ：中间值定理Interval ：区间Inverse function ：反函数Inverse trigonometric function ：反三角函数Iterated integral ：逐次积分H:Higher mathematics 高等数学/高数E、F、G、H:Ellipse ：椭圆Ellipsoid ：椭圆体Epicycloid ：外摆线Equation ：方程式Even function ：偶函数Expected Valued ：期望值Exponential Function ：指数函数Exponents , laws of ：指数率Extreme value ：极值Extreme Value Theorem ：极值定理Factorial ：阶乘First Derivative Test ：一阶导数试验法First octant ：第一卦限Focus ：焦点Fractions ：分式Function ：函数Fundamental Theorem of Calculus ：微积分基本定理Geometric series ：几何级数Gradient ：梯度Graph ：图形Green Formula ：格林公式Half-angle formulas ：半角公式Harmonic series ：调和级数Helix ：螺旋线Higher Derivative ：高阶导数Horizontal asymptote ：水平渐近线。

高等数学名词(中英文)

高等数学名词(中英文)第一章函数与极限Chapter1 Function and Limit 集合set元素element子集subset空集empty set并集union交集intersection差集difference of set基本集basic set补集complement set直积direct product笛卡儿积Cartesian product开区间open interval闭区间closed interval半开区间half open interval有限区间finite interval区间的长度length of an interval无限区间infinite interval领域neighborhood领域的中心centre of a neighborhood 领域的半径radius of a neighborhood 左领域left neighborhood右领域right neighborhood映射mappingX到Y的映射mapping of X ontoY 满射surjection单射injection一一映射one-to-one mapping双射bijection算子operator变化transformation函数function逆映射inverse mapping复合映射composite mapping自变量independent variable 因变量dependent variable定义域domain函数值value of function函数关系function relation值域range自然定义域natural domain单值函数single valued function多值函数multiple valued function单值分支one-valued branch函数图形graph of a function绝对值函数absolute value符号函数sigh function整数部分integral part阶梯曲线step curve当且仅当if and only if(iff)分段函数piecewise function上界upper bound下界lower bound有界boundedness无界unbounded函数的单调性monotonicity of a function 单调增加的increasing单调减少的decreasing单调函数monotone function函数的奇偶性parity(odevity) of a function 对称symmetry偶函数even function奇函数odd function函数的周期性periodicity of a function周期period反函数inverse function直接函数direct function复合函数composite function中间变量intermediate variable函数的运算operation of function基本初等函数basic elementary function初等函数elementary function幂函数power function指数函数exponential function对数函数logarithmic function三角函数trigonometric function反三角函数inverse trigonometric function常数函数constant function双曲函数hyperbolic function双曲正弦hyperbolic sine双曲余弦hyperbolic cosine双曲正切hyperbolic tangent反双曲正弦inverse hyperbolic sine反双曲余弦inverse hyperbolic cosine反双曲正切inverse hyperbolic tangent极限limit数列sequence of number收敛convergence收敛于a converge to a发散divergent极限的唯一性uniqueness of limits收敛数列的有界性boundedness of a convergent sequence子列subsequence函数的极限limits of functions函数当x趋于x0时的极限limit of functions as x approaches x0左极限left limit右极限right limit单侧极限one-sided limits水平渐近线horizontal asymptote无穷小infinitesimal无穷大infinity铅直渐近线vertical asymptote夹逼准则squeeze rule单调数列monotonic sequence高阶无穷小infinitesimal of higher order低阶无穷小infinitesimal of lower order同阶无穷小infinitesimal of the same order等阶无穷小equivalent infinitesimal 函数的连续性continuity of a function增量increment函数在x0连续the function is continuous at x0左连续left continuous右连续right continuous区间上的连续函数continuous function函数在该区间上连续function is continuous on an interval不连续点discontinuity point第一类间断点discontinuity point of the first kind第二类间断点discontinuity point of the second kind初等函数的连续性continuity of the elementary functions定义区间defined interval最大值global maximum value (absolute maximum)最小值global minimum value (absolute minimum)零点定理the zero point theorem介值定理intermediate value theorem第二章导数与微分Chapter2 Derivative and Differential速度velocity匀速运动uniform motion平均速度average velocity瞬时速度instantaneous velocity圆的切线tangent line of a circle切线tangent line切线的斜率slope of the tangent line位置函数position function导数derivative可导derivable函数的变化率问题problem of the change rate of a function导函数derived function左导数left-hand derivative右导数right-hand derivative单侧导数one-sided derivatives在闭区间[a, b]上可导is derivable on the closed interval [a,b]切线方程tangent equation角速度angular velocity成本函数cost function边际成本marginal cost链式法则chain rule隐函数implicit function显函数explicit function二阶函数second derivative三阶导数third derivative高阶导数nth derivative莱布尼茨公式Leibniz formula对数求导法log- derivative参数方程parametric equation相关变化率correlative change rata微分differential可微的differentiable函数的微分differential of function自变量的微分differential of independent variable微商differential quotient间接测量误差indirect measurement error绝对误差absolute error相对误差relative error第三章微分中值定理与导数的应用Chapter3 Mean Value Theorem ofDifferentials and the Application ofDerivatives罗马定理Rolle’s theorem费马引理Fermat’s lemma拉格朗日中值定理Lagrange’s mean value theorem驻点stationary point稳定点stable point 临界点critical point辅助函数auxiliary function拉格朗日中值公式Lagrange’s mean value formula柯西中值定理Cauchy’s mean value theorem 洛必达法则L’Hospital’s Rule0/0型不定式indeterminate form of type 0/0 不定式indeterminate form泰勒中值定理Taylor’s mean value theorem 泰勒公式Taylor formula余项remainder term拉格朗日余项Lagrange remainder term麦克劳林公式Maclaurin’s formula佩亚诺公式Peano remainder term凹凸性concavity凹向上的concave upward, cancave up凹向下的，向上凸的concave downward’ concave down拐点inflection point函数的极值extremum of function极大值local(relative) maximum最大值global(absolute) mximum极小值local(relative) minimum最小值global(absolute) minimum目标函数objective function曲率curvature弧微分arc differential平均曲率average curvature曲率园circle of curvature曲率中心center of curvature曲率半径radius of curvature渐屈线evolute渐伸线involute根的隔离isolation of root隔离区间isolation interval切线法tangent line method第四章不定积分Chapter4 Indefinite Integrals原函数primitive function(anti-derivative)积分号sign of integration被积函数integrand积分变量integral variable积分曲线integral curve积分表table of integrals换元积分法integration by substitution分部积分法integration by parts分部积分公式formula of integration by parts 有理函数rational function真分式proper fraction假分式improper fraction第五章定积分Chapter5 Definite Integrals曲边梯形trapezoid with曲边curve edge窄矩形narrow rectangle曲边梯形的面积area of trapezoid with curved edge积分下限lower limit of integral积分上限upper limit of integral积分区间integral interval分割partition积分和integral sum可积integrable矩形法rectangle method积分中值定理mean value theorem of integrals函数在区间上的平均值average value of a function on an integvals牛顿－莱布尼茨公式Newton-Leibniz formula微积分基本公式fundamental formula of calculus换元公式formula for integration by substitution递推公式recurrence formula反常积分improper integral 反常积分发散the improper integral is divergent反常积分收敛the improper integral is convergent无穷限的反常积分improper integral on an infinite interval无界函数的反常积分improper integral of unbounded functions绝对收敛absolutely convergent第六章定积分的应用Chapter6 Applications of the DefiniteIntegrals元素法the element method面积元素element of area平面图形的面积area of a luane figure直角坐标又称“笛卡儿坐标(Cartesian coordinates)”极坐标polar coordinates抛物线parabola椭圆ellipse旋转体的面积volume of a solid of rotation旋转椭球体ellipsoid of revolution, ellipsoid of rotation曲线的弧长arc length of acurve可求长的rectifiable光滑smooth功work水压力water pressure引力gravitation变力variable force第七章空间解析几何与向量代数Chapter7 Space Analytic Geometry andVector Algebra向量vector自由向量free vector单位向量unit vector零向量zero vector相等equal平行parallel向量的线性运算linear poeration of vector三角法则triangle rule平行四边形法则parallelogram rule交换律commutative law结合律associative law负向量negative vector差difference分配律distributive law空间直角坐标系space rectangular coordinates坐标面coordinate plane卦限octant向量的模modulus of vector向量a与b的夹角angle between vector a and b方向余弦direction cosine方向角direction angle向量在轴上的投影projection of a vector onto an axis数量积，外积，叉积scalar product,dot product,inner product曲面方程equation for a surface球面sphere旋转曲面surface of revolution母线generating line轴axis圆锥面cone顶点vertex旋转单叶双曲面revolution hyperboloids of one sheet旋转双叶双曲面revolution hyperboloids of two sheets柱面cylindrical surface ,cylinder圆柱面cylindrical surface准线directrix抛物柱面parabolic cylinder二次曲面quadric surface 椭圆锥面dlliptic cone椭球面ellipsoid单叶双曲面hyperboloid of one sheet双叶双曲面hyperboloid of two sheets旋转椭球面ellipsoid of revolution椭圆抛物面elliptic paraboloid旋转抛物面paraboloid of revolution双曲抛物面hyperbolic paraboloid马鞍面saddle surface椭圆柱面elliptic cylinder双曲柱面hyperbolic cylinder抛物柱面parabolic cylinder空间曲线space curve空间曲线的一般方程general form equations of a space curve空间曲线的参数方程parametric equations of a space curve螺转线spiral螺矩pitch投影柱面projecting cylinder投影projection平面的点法式方程pointnorm form eqyation of a plane法向量normal vector平面的一般方程general form equation of a plane两平面的夹角angle between two planes点到平面的距离distance from a point to a plane空间直线的一般方程general equation of a line in space方向向量direction vector直线的点向式方程pointdirection form equations of a line方向数direction number直线的参数方程parametric equations of a line两直线的夹角angle between two lines垂直perpendicular直线与平面的夹角angle between a line and a planes平面束pencil of planes平面束的方程equation of a pencil of planes 行列式determinant系数行列式coefficient determinant第八章多元函数微分法及其应用Chapter8 Differentiation of Functions ofSeveral Variables and Its Application一元函数function of one variable多元函数function of several variables内点interior point外点exterior point边界点frontier point,boundary point聚点point of accumulation开集openset闭集closed set连通集connected set开区域open region闭区域closed region有界集bounded set无界集unbounded setn维空间n-dimentional space二重极限double limit多元函数的连续性continuity of function of seveal连续函数continuous function不连续点discontinuity point一致连续uniformly continuous偏导数partial derivative对自变量x的偏导数partial derivative with respect to independent variable x高阶偏导数partial derivative of higher order 二阶偏导数second order partial derivative混合偏导数hybrid partial derivative全微分total differential偏增量oartial increment偏微分partial differential 全增量total increment可微分differentiable必要条件necessary condition充分条件sufficient condition叠加原理superpostition principle全导数total derivative中间变量intermediate variable隐函数存在定理theorem of the existence of implicit function曲线的切向量tangent vector of a curve法平面normal plane向量方程vector equation向量值函数vector-valued function切平面tangent plane法线normal line方向导数directional derivative梯度gradient数量场scalar field梯度场gradient field向量场vector field势场potential field引力场gravitational field引力势gravitational potential曲面在一点的切平面tangent plane to a surface at a point曲线在一点的法线normal line to a surface at a point无条件极值unconditional extreme values条件极值conditional extreme values拉格朗日乘数法Lagrange multiplier method 拉格朗日乘子Lagrange multiplier经验公式empirical formula最小二乘法method of least squares均方误差mean square error第九章重积分Chapter9 Multiple Integrals二重积分double integral可加性additivity累次积分iterated integral体积元素volume element三重积分triple integral直角坐标系中的体积元素volume element in rectangular coordinate system柱面坐标cylindrical coordinates柱面坐标系中的体积元素volume element in cylindrical coordinate system球面坐标spherical coordinates球面坐标系中的体积元素volume element in spherical coordinate system反常二重积分improper double integral曲面的面积area of a surface质心centre of mass静矩static moment密度density形心centroid转动惯量moment of inertia参变量parametric variable第十章曲线积分与曲面积分Chapter10 Line (Curve) Integrals andSurface Integrals对弧长的曲线积分line integrals with respect to arc hength第一类曲线积分line integrals of the first type对坐标的曲线积分line integrals with respect to x,y,and z第二类曲线积分line integrals of the second type有向曲线弧directed arc单连通区域simple connected region复连通区域complex connected region格林公式Green formula第一类曲面积分surface integrals of the first type对面的曲面积分surface integrals with respect to area 有向曲面directed surface对坐标的曲面积分surface integrals with respect to coordinate elements第二类曲面积分surface integrals of the second type有向曲面元element of directed surface高斯公式gauss formula拉普拉斯算子Laplace operator格林第一公式Green’s first formula通量flux散度divergence斯托克斯公式Stokes formula环流量circulation旋度rotation,curl第十一章无穷级数Chapter11 Infinite Series一般项general term部分和partial sum余项remainder term等比级数geometric series几何级数geometric series公比common ratio调和级数harmonic series柯西收敛准则Cauchy convergence criteria, Cauchy criteria for convergence正项级数series of positive terms达朗贝尔判别法D’Alembert test柯西判别法Cauchy test交错级数alternating series绝对收敛absolutely convergent条件收敛conditionally convergent柯西乘积Cauchy product函数项级数series of functions发散点point of divergence收敛点point of convergence收敛域convergence domain和函数sum function幂级数power series幂级数的系数coeffcients of power series阿贝尔定理Abel Theorem收敛半径radius of convergence收敛区间interval of convergence泰勒级数Taylor series麦克劳林级数Maclaurin series二项展开式binomial expansion近似计算approximate calculation舍入误差round-off error,rounding error欧拉公式Euler’s formula魏尔斯特拉丝判别法Weierstrass test三角级数trigonometric series振幅amplitude角频率angular frequency初相initial phase矩形波square wave谐波分析harmonic analysis直流分量direct component基波fundamental wave二次谐波second harmonic三角函数系trigonometric function system傅立叶系数Fourier coefficient傅立叶级数Forrier series周期延拓periodic prolongation正弦级数sine series余弦级数cosine series奇延拓odd prolongation偶延拓even prolongation傅立叶级数的复数形式complex form of Fourier series第十二章微分方程Chapter12 Differential Equation解微分方程solve a differential equation常微分方程ordinary differential equation偏微分方程partial differential equation,PDE 微分方程的阶order of a differential equation 微分方程的解solution of a differential equation 微分方程的通解general solution of a differential equation初始条件initial condition微分方程的特解particular solution of a differential equation初值问题initial value problem微分方程的积分曲线integral curve of a differential equation可分离变量的微分方程variable separable differential equation隐式解implicit solution隐式通解inplicit general solution衰变系数decay coefficient衰变decay齐次方程homogeneous equation一阶线性方程linear differential equation of first order非齐次non-homogeneous齐次线性方程homogeneous linear equation 非齐次线性方程non-homogeneous linear equation常数变易法method of variation of constant 暂态电流transient state current稳态电流steady state current伯努利方程Bernoulli equation全微分方程total differential equation积分因子integrating factor高阶微分方程differential equation of higher order悬链线catenary高阶线性微分方程linear differential equation of higher order自由振动的微分方程differential equation of free vibration强迫振动的微分方程differential equation of forced oscillation串联电路的振荡方程oscillation equation of series circuit二阶线性微分方程second order lineardifferential equation线性相关linearly dependence线性无关linearly independence二阶常系数齐次线性微分方程second order homogeneous linear differential equation with constant coefficient二阶变系数齐次线性微分方程second order homogeneous linear differential equation with variable coefficient特征方程characteristic equation无阻尼自由振动的微分方程differential equation of free vibration with zero damping 固有频率natural frequency 简谐振动simple harmonic oscillation,simple harmonic vibration微分算子differential operator待定系数法method of undetermined coefficient共振现象resonance phenomenon欧拉方程Euler equation幂级数解法power series solution数值解法numerial solution勒让德方程Legendre equation微分方程组system of differential equations常系数线性微分方程组system of linear differential equations with constant coefficientV、X、Z:Value of function ：函数值Variable ：变数Vector ：向量Velocity ：速度Vertical asymptote ：垂直渐近线Volume ：体积X-axis ：x轴x-coordinate ：x坐标x-intercept ：x截距Zero vector ：函数的零点Zeros of a polynomial ：多项式的零点T:Tangent function ：正切函数Tangent line ：切线Tangent plane ：切平面Tangent vector ：切向量Total differential ：全微分Trigonometric function ：三角函数Trigonometric integrals ：三角积分Trigonometric substitutions ：三角代换法Tripe integrals ：三重积分S:Saddle point ：鞍点Scalar ：纯量Secant line ：割线Second derivative ：二阶导数Second Derivative Test ：二阶导数试验法Second partial derivative ：二阶偏导数Sector ：扇形Sequence ：数列Series ：级数Set ：集合Shell method ：剥壳法Sine function ：正弦函数Singularity ：奇点Slant asymptote ：斜渐近线Slope ：斜率Slope-intercept equation of a line ：直线的斜截式Smooth curve ：平滑曲线Smooth surface ：平滑曲面Solid of revolution ：旋转体Space ：空间Speed ：速率Spherical coordinates ：球面坐标Squeeze Theorem ：夹挤定理Step function ：阶梯函数Strictly decreasing ：严格递减Strictly increasing ：严格递增Sum ：和Surface ：曲面Surface integral ：面积分Surface of revolution ：旋转曲面Symmetry ：对称R:Radius of convergence ：收敛半径Range of a function ：函数的值域Rate of change ：变化率Rational function ：有理函数Rationalizing substitution ：有理代换法Rational number ：有理数Real number ：实数Rectangular coordinates ：直角坐标Rectangular coordinate system ：直角坐标系Relative maximum and minimum ：相对极大值与极小值Revenue function ：收入函数Revolution , solid of ：旋转体Revolution , surface of ：旋转曲面Riemann Sum ：黎曼和Riemannian geometry ：黎曼几何Right-hand derivative ：右导数Right-hand limit ：右极限Root ：根P、Q:Parabola ：拋物线Parabolic cylinder ：抛物柱面Paraboloid ：抛物面Parallelepiped ：平行六面体Parallel lines ：并行线Parameter ：参数Partial derivative ：偏导数Partial differential equation ：偏微分方程Partial fractions ：部分分式Partial integration ：部分积分Partiton ：分割Period ：周期Periodic function ：周期函数Perpendicular lines ：垂直线Piecewise defined function 分段定义函数Plane ：平面Point of inflection ：反曲点Polar axis ：极轴Polar coordinate ：极坐标Polar equation ：极方程式Pole ：极点Polynomial ：多项式Positive angle ：正角Point-slope form ：点斜式Power function ：幂函数Product ：积Quadrant ：象限Quotient Law of limit 极限的商定律Quotient Rule ：商定律M、N、O:Maximum and minimum values ：极大与极小值Mean Value Theorem ：均值定理Multiple integrals ：重积分Multiplier ：乘子Natural exponential function 自然指数函数Natural logarithm function 自然对数函数Natural number ：自然数Normal line ：法线Normal vector ：法向量Number ：数Octant ：卦限Odd function ：奇函数One-sided limit ：单边极限Open interval ：开区间Optimization problems ：最佳化问题Order ：阶Ordinary differential equation ：常微分方程Origin ：原点Orthogonal ：正交的L:Laplace transform ：Leplace 变换Law of Cosines ：余弦定理Least upper bound ：最小上界Left-hand derivative ：左导数Left-hand limit ：左极限Lemniscate ：双钮线Length ：长度Level curve ：等高线L'Hospital's rule 洛必达法则Limacon ：蚶线Limit ：极限Linear approximation：线性近似Linear equation ：线性方程式Linear function ：线性函数Linearity ：线性Linearization ：线性化Line in the plane ：平面上之直线Line in space ：空间之直线Lobachevski geometry 罗巴切夫斯基几何Local extremum ：局部极值Local maximum and minimum 局部极大值与极小值Logarithm ：对数Logarithmic function ：对数函数I:Implicit differentiation ：隐求导法Implicit function ：隐函数Improper integral ：瑕积分Increasing/Decreasing Test 递增或递减试验法Increment ：增量Increasing Function ：增函数Indefinite integral ：不定积分Independent variable ：自变数Indeterminate from ：不定型Inequality ：不等式Infinite point ：无穷极限Infinite series ：无穷级数Inflection point ：反曲点Instantaneous velocity ：瞬时速度Integer ：整数Integral ：积分Integrand ：被积分式Integration ：积分Integration by part ：分部积分法Intercepts ：截距Intermediate value of Theorem 中间值定理Interval ：区间Inverse function ：反函数Inverse trigonometric function反三角函数Iterated integral ：逐次积分H:Higher mathematics 高等数学/高数E、F、G、H:Ellipse ：椭圆Ellipsoid ：椭圆体Epicycloid ：外摆线Equation ：方程式Even function ：偶函数Expected Valued ：期望值Exponential Function ：指数函数Exponents , laws of ：指数率Extreme value ：极值Extreme Value Theorem ：极值定理Factorial ：阶乘First Derivative Test ：一阶导数试验法First octant ：第一卦限Focus ：焦点Fractions ：分式Function ：函数Fundamental Theorem of Calculus ：微积分基本定理Geometric series ：几何级数Gradient ：梯度Graph ：图形Green Formula ：格林公式Half-angle formulas ：半角公式Harmonic series ：调和级数Helix ：螺旋线Higher Derivative ：高阶导数Horizontal asymptote ：水平渐近线Horizontal line ：水平线Hyperbola ：双曲线Hyper boloid ：双曲面D:Decreasing function ：递减函数Decreasing sequence ：递减数列Definite integral ：定积分Degree of a polynomial ：多项式之次数Density ：密度Derivative ：导数of a composite function ：复合函数之导数of a constant function ：常数函数之导数directional ：方向导数domain of ：导数之定义域of exponential function ：指数函数之导数higher ：高阶导数partial ：偏导数of a power function ：幂函数之导数of a power series ：羃级数之导数of a product ：积之导数of a quotient ：商之导数as a rate of change ：导数当作变率right-hand ：右导数second ：二阶导数as the slope of a tangent 导数看成切线之斜率Determinant ：行列式Differentiable function 可导函数Differential ：微分Differential equation ：微分方程partial ：偏微分方程Differentiation ：求导法implicit ：隐求导法partial ：偏微分法term by term ：逐项求导法Directional derivatives ：方向导数Discontinuity ：不连续性Disk method ：圆盘法Distance ：距离Divergence ：发散Domain ：定义域Dot product ：点积Double integral ：二重积分change of variable in二重积分之变数变换in polar coordinates ：极坐标二重积分C:Calculus ：微积分differential ：微分学integral ：积分学Cartesian coordinates ：笛卡儿坐标一般指直角坐标Cartesian coordinates system 笛卡儿坐标系Cauch’s Mean Value Theorem 柯西均值定理Chain Rule ：连锁律Change of variables ：变数变换Circle ：圆Circular cylinder ：圆柱Closed interval ：封闭区间Coefficient ：系数Composition of function ：函数之合成Compound interest ：复利Concavity ：凹性Conchoid ：蚌线Cone ：圆锥Constant function ：常数函数Constant of integration ：积分常数Continuity ：连续性at a point ：在一点处之连续性of a function ：函数之连续性on an interval ：在区间之连续性from the left ：左连续from the right ：右连续Continuous function ：连续函数Convergence ：收敛interval of ：收敛区间radius of ：收敛半径Convergent sequence ：收敛数列series ：收敛级数Coordinate：s：坐标Cartesian ：笛卡儿坐标cylindrical ：柱面坐标polar ：极坐标rectangular ：直角坐标spherical ：球面坐标Coordinate axes ：坐标轴Coordinate planes ：坐标平面Cosine function ：余弦函数Critical point ：临界点Cubic function ：三次函数Curve ：曲线Cylinder：圆柱Cylindrical Coordinates ：圆柱坐标A、B:Absolute convergence ：绝对收敛Absolute extreme values ：绝对极值Absolute maximum and minimum 绝对极大与极小Absolute value ：绝对值Absolute value function ：绝对值函数Acceleration ：加速度Antiderivative ：反导数Approximate integration ：近似积分Approximation ：逼近法by differentials ：用微分逼近linear ：线性逼近法by Simpson’s Rule ：Simpson法则逼近法by the Trapezoidal Rule 梯形法则逼近法Arbitrary constant ：任意常数Arc length ：弧长Area ：面积under a curve ：曲线下方之面积between curves ：曲线间之面积in polar coordinates ：极坐标表示之面积of a sector of a circle ：扇形之面积of a surface of a revolution 旋转曲面之面积Asymptote ：渐近线horizontal ：水平渐近线slant ：斜渐近线vertical ：垂直渐近线Average speed ：平均速率Average velocity ：平均速度Axes, coordinate ：坐标轴Axes of ellipse ：椭圆之轴Binomial series ：二项级数微积分专有名词中英文对照absolutely convergent 绝对收敛absolute value 绝对值algebraic function 代数函数analytic geometry 解析几何antiderivative 不定积分approximate integration 近似积分approximation 近似法、逼近法arbitrary constant 任意常数arithmetic series/progression (AP)算数级数asymptotes (vertical and horizontal)(垂直/水平)渐近线average rate of change 平均变化率base 基数binomial theorem 二项式定理，二项展开式Cartesian coordinates 笛卡儿坐标(一般指直角坐标)Cartesian coordinates system 笛卡儿坐标系Cauch’s Mean Value Theorem 柯西均值定理chain rule 链式求导法则calculus 微积分学closed interval integral 闭区间积分coefficient 系数composite function 复合函数conchoid 蚌线continuity (函数的)连续性concavity (函数的)凹凸性conditionally convergent 有条件收敛continuity 连续性critical point 临界点cubic function 三次函数cylindrical coordinates 圆柱坐标decreasing function 递减函数decreasing sequence 递减数列definite integral 定积分derivative 导数determinant 行列式differential coefficient 微分系数differential equation 微分方程directional derivative 方向导数discontinuity 不连续性discriminant (二次函数)判别式disk method 圆盘法divergence 散度divergent 发散的domain 定义域dot product 点积double integral 二重积分ellipse 椭圆ellipsoid 椭圆体epicycloid 外摆线Euler's method (BC)欧拉法expected valued 期望值exponential function 指数函数extreme value heorem 极值定理factorial 阶乘finite series 有限级数fundamental theorem of calculus 微积分基本定理geometric series/progression (GP)几何级数gradient 梯度Green formula 格林公式half-angle formulas 半角公式harmonic series 调和级数helix 螺旋线higher derivative 高阶导数horizontal asymptote 水平渐近线horizontal line 水平线hyperbola 双曲线hyper boloid 双曲面implicit differentiation 隐函数求导implicit function 隐函数improper integral 广义积分、瑕积分increment 增量increasing function 增函数indefinite integral 不定积分independent variable 自变数inequality 不等式ndeterminate form 不定型infinite point 无穷极限infinite series 无穷级数infinite series 无限级数inflection point (POI) 拐点initial condition 初始条件instantaneous rate of change 瞬时变化率integrable 可积的integral 积分integrand 被积分式integration 积分integration by part 分部积分法intercept 截距intermediate value of Theorem ：中间值定理inverse function 反函数irrational function 无理函数iterated integral 逐次积分Laplace transform 拉普拉斯变换law of cosines 余弦定理least upper bound 最小上界left-hand derivative 左导数left-hand limit 左极限L'Hospital's rule 洛必达法则limacon 蚶线linear approximation 线性近似法linear equation 线性方程式linear function 线性函数linearity 线性linearization 线性化local maximum 极大值local minimum 极小值logarithmic function 对数函数MacLaurin series 麦克劳林级数maximum 最大值mean value theorem (MVT)中值定理minimum 最小值method of lagrange multipliers 拉格朗日乘数法modulus 绝对值multiple integral 多重积分multiple 倍数multiplier 乘子octant 卦限open interval integral 开区间积分optimization 优化法，极值法origin 原点orthogonal 正交parametric equation (BC)参数方程partial derivative 偏导数partial differential equation 偏微分方程partial fractions 部分分式piece-wise function 分段函数parabola 抛物线parabolic cylinder 抛物柱面paraboloid ：抛物面parallelepiped 平行六面体parallel lines 并行线parameter ：参数partial integration 部分积分partiton ：分割period ：周期periodic function 周期函数perpendicular lines 垂直线piecewise defined function 分段定义函数plane 平面point of inflection 反曲点point-slope form 点斜式polar axis 极轴polar coordinates 极坐标polar equation 极坐标方程pole 极点polynomial 多项式power series 幂级数product rule 积的求导法则quadrant 象限quadratic functions 二次函数quotient rule 商的求导法则radical 根式radius of convergence 收敛半径range 值域(related) rate of change with time (时间)变化率rational function 有理函数reciprocal 倒数remainder theorem 余数定理Riemann sum 黎曼和Riemannian geometry 黎曼几何right-hand limit 右极限Rolle's theorem 罗尔(中值)定理root 根rotation 旋转secant line 割线second derivative 二阶导数second derivative test 二阶导数试验法second partial derivative 二阶偏导数series 级数shell method (积分)圆筒法sine function 正弦函数singularity 奇点slant 母线slant asymptote 斜渐近线slope 斜率slope-intercept equation of a line 直线的斜截式smooth curve 平滑曲线smooth surface 平滑曲面solid of revolution 旋转体symmetry 对称性substitution 代入法、变量代换tangent function 正切函数tangent line 切线tangent plane 切(平)面tangent vector 切矢量taylor's series 泰勒级数three-dimensional analytic geometry 空间解析几何total differentiation 全微分trapezoid rule 梯形(积分)法则。

数学专业英语(Doc版).Word5

数学专业英语－Differential CalculusHistorical IntroductionNewton and Leibniz,quite independently of one another,were largely responsible for developing the ideas of integral calculus to the point where hitherto insur mountable problems could be solved by more or less routine methods.The succ essful accomplishments of these men were primarily due to the fact that they were able to fuse together the integral calculus with the second main branch o f calculus,differential calculus.The central idea of differential calculus is the notion of derivative.Like the inte gral,the derivative originated from a problem in geometry—the problem of find ing the tangent line at a point of a curve.Unlile the integral,however,the deriva tive evolved very late in the history of mathematics.The concept was not form ulated until early in the 17th century when the French mathematician Pierre de Fermat,attempted to determine the maxima and minima of certain special func tions.Fermat’s idea,basically very simple,can be understood if we refer to a curve a nd assume that at each of its points this curve has a definite direction that ca n be described by a tangent line.Fermat noticed that at certain points where th e curve has a maximum or minimum,the tangent line must be horizontal.Thus t he problem of locating such extreme values is seen to depend on the solution of another problem,that of locating the horizontal tangents.This raises the more general question of determining the direction of the tange nt line at an arbitrary point of the curve.It was the attempt to solve this gener al problem that led Fermat to discover some of the rudimentary ideas underlyi ng the notion of derivative.At first sight there seems to be no connection whatever between the problem of finding the area of a region lying under a curve and the problem of findin g the tangent line at a point of a curve.The first person to realize that these t wo seemingly remote ideas are,in fact, rather intimately related appears to have been Newton’s teacher,Isaac Barrow(1630-1677).However,Newton and Leibniz were the first to understand the real importance of this relation and they explo ited it to the fullest,thus inaugurating an unprecedented era in the development of mathematics.Although the derivative was originally formulated to study the problem of tang ents,it was soon found that it also provides a way to calculate velocity and,mo re generally,the rate of change of a function.In the next section we shall consi der a special problem involving the calculation of a velocity.The solution of this problem contains all the essential fcatures of the derivative concept and may help to motivate the general definition of derivative which is given below.A Problem Involving VelocitySuppose a projectile is fired straight up from the ground with initial velocity o f 144 feet persecond.Neglect friction,and assume the projectile is influenced onl y by gravity so that it moves up and back along a straight line.Let f(t) denote the height in feet that the projectile attains t seconds after firing.If the force of gravity were not acting on it,the projectile would continue to move upward with a constant velocity,traveling a distance of 144 feet every second,and at ti me t we woule have f(t)=144 t.In actual practice,gravity causes the projectile t o slow down until its velocity decreases to zero and then it drops back to eart h.Physical experiments suggest that as the projectile is aloft,its height f(t) is gi ven by the formula(1)f(t)=144t –16 t2The term –16t2is due to the influence of gravity.Note that f(t)=0 when t=0 a nd when t=9.This means that the projectile returns to earth after 9 seconds and it is to be understood that formula (1) is valid only for 0<t<9.The problem we wish to consider is this:To determine the velocity of the proj ectile at each instant of its motion.Before we can understand this problem,we must decide on what is meant by the velocity at each instant.To do this,we int roduce first the notion of average velocity during a time interval,say from time t to time t+h.This is defined to be the quotient.Change in distance during time interval =f(t+h)-f(t)/hThis quotient,called a difference quotient,is a number which may be calculated whenever both t and t+h are in the interval[0,9].The number h may be positiv e or negative,but not zero.We shall keep t fixed and see what happens to the difference quotient as we take values of h with smaller and smaller absolute v alue.The limit process by which v(t) is obtained from the difference quotient is wri tten symbolically as follows:V(t)=lim(h→0)[f(t+h)-f(t)]/hThe equation is used to define velocity not only for this particular example bu t,more generally,for any particle moving along a straight line,provided the position function f is such that the differerce quotient tends to a definite limit as h approaches zero.The example describe in the foregoing section points the way to the introducti on of the concept of derivative.We begin with a function f defined at least on some open interval(a,b) on the x axis.Then we choose a fixed point in this in terval and introduce the difference quotient[f(x+h)-f(x)]/hwhere the number h,which may be positive or negative(but not zero),is such th at x+h also lies in(a,b).The numerator of this quotient measures the change in the function when x changes from x to x+h.The quotient itself is referred to a s the average rate of change of f in the interval joining x to x+h.Now we let h approach zero and see what happens to this quotient.If the quot ient.If the quotient approaches some definite values as a limit(which implies th at the limit is the same whether h approaches zero through positive values or through negative values),then this limit is called the derivative of f at x and is denoted by the symbol f’(x) (read as “f prime of x”).Thus the formal defi nition of f’(x) may be stated as follows:Definition of derivative.The derivative f’(x)is defined by the equationf’(x)=lim(h→o)[f(x+h)-f(x)]/hprovided the limit exists.The number f’(x) is also called the rate of change of f at x.In general,the limit process which produces f’(x) from f(x) gives a way of ob taining a new function f’from a given function f.This process is called differ entiation,and f’is called the first derivative of f.If f’,in turn,is defined on an interval,we can try to compute its first derivative,denoted by f’’,and is calle d the second derivative of f.Similarly,the nth derivative of f denoted by f^(n),is defined to be the first derivative of f^(n-1).We make the convention that f^(0) =f,that is,the zeroth derivative is the function itself.Vocabularydifferential calculus微积分differentiable可微的intergral calculus 积分学differentiate 求微分hither to 迄今 integration 积分法insurmountable 不能超越 integral 积分routine 惯常的integrable 可积的fuse 融合integrate 求积分originate 起源于sign-preserving保号evolve 发展，引出 axis 轴（单数）tangent line 切线 axes 轴（复数）direction 方向 contradict 矛盾horizontal 水平的contradiction 矛盾vertical 垂直的 contrary 相反的rudimentary 初步的，未成熟的composite function 合成函数，复合函数area 面积composition 复合函数intimately 紧密地interior 内部exploit 开拓，开发 interior point 内点inaugurate 开始 imply 推出，蕴含projectile 弹丸 aloft 高入云霄friction摩擦initial 初始的gravity 引力 instant 瞬时rate of change 变化率integration by parts分部积分attain 达到definite integral 定积分defferential 微分indefinite integral 不定积分differentiation 微分法 average 平均Notes1. Newton and Leibniz,quite independently of one another,were largely responsible for developing…by more or less routine methods.意思是：在很大程度上是牛顿和莱伯尼，他们相互独立地把积分学的思想发展到这样一种程度，使得迄今一些难于超越的问题可以或多或少地用通常的方法加以解决。

数学专业英语翻译

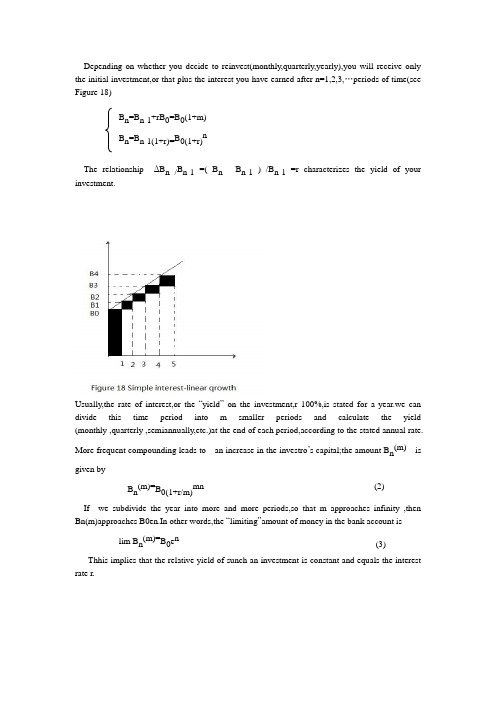
Depending on whether you decide to reinvest(monthly,quarterly,yearly),you will receive only the initial investment,or that plus the interest you have earned after n=1,2,3,…periods of time(see Figure 18)B n=B n-1+rB0=B0(1+m)B n=B n-1(1+r)=B0(1+r)nThe relationship ΔB n /B n-1 =( B n- B n-1 ) /B n-1 =r characterizes the yield of your investment.Usually,the rate of interest,or the “yield”on the investment,r 100%,is stated for a year.we can divide this time period into m smaller periods and calculate the yield (monthly ,quarterly ,semiannually,etc.)at the end of each period,according to the stated annual rate.More frequent compounding leads to an increase in the investro’s capital;the amount B n(m)is given byB n(m)=B0(1+r/m)mn (2)If we subdivide the year into more and more periods,so that m approaches infinity ,then Bn(m)approaches B0en.In other words,the “limiting”amount of money in the bank account islim B n(m)=B0e n(3)Thhis implies that the relative yield of sunch an investment is constant and equals the interest rate r.The three methods of calculating interest discussed above are called simple ,compound ,and continuous .Formulas (1),(2)and (3)provide ways to calculate the amount in the investor’s bank account and clearly show the dependence of the value of money on time.On the other hand,we have the bank’s interest rate R,s that if we invest the amount (1-R)B1,at time 1 ,say after one year,we will receive the amount B1.This is equivalent to the issuance of a bond with a nominal value B1(to be paid to the bond holder at the end of this year),but now the bond sells for a lower price(decreased by the amount of the lending rate R over one period ,that is m=1).So today’s price is determined by the formula(1-R)B1,which is equal to the discounted price B1/(1+r) .Therefore,we can view the bank account as a coupon-free bond in the sense of a risk-free asset of the financial market.The lack of ,or very small,changes in interest rates characterize the stability of financial and economic systems ,for which the corresponding bank acccount serves as the basic non-risky asset.Reality shows that such suggestions present limits in The idealization of mathematical models for financial markets.译文：依赖于你是否决定再投资（平稳的，按季度的，一年一次的），你将仅仅收到最初的投资，否则就会加上n=1,2,3…个时期后你所赚的利息。

数学专业英语论文(含中文版)

Differential CalculusNewton and Leibniz,quite independently of one another,were largely responsible for developing the ideas of integral calculus to the point where hitherto insurmountable problems could be solved by more or less routine methods.The successful accomplishments of these men were primarily due to the fact that they were able to fuse together the integral calculus with the second main branch of calculus,differential calculus.In this article, we give su ﬃcient conditions for controllability of some partial neutral functional di ﬀerential equations with inﬁnite delay. We suppose that the linear part is not necessarily densely deﬁned but satisﬁes the resolvent estimates of the Hille -Yosida theorem. The results are obtained using the integrated semigroups theory. An application is given to illustrate our abstract result. Key words Controllability; integrated semigroup; integral solution; inﬁnity delay1 IntroductionIn this article, we establish a result about controllability to the following class of partial neutral functional di ﬀerential equations with inﬁnite delay:0,),()(0≥⎪⎩⎪⎨⎧∈=++=∂∂t x xt t F t Cu ADxt Dxt tβφ (1) where the state variable (.)x takes values in a Banach space ).,(E and the control (.)u is given in []0),,,0(2>T U T L ,the Banach space of admissible control functions with U a Banach space. Cis a bounded linear operator from U into E, A : D(A) ⊆ E → E is a linear operator on E, B is the phase space of functions mapping (−∞, 0] into E, which will be speciﬁed later, D is a bounded linear operator from B into E deﬁned byB D D ∈-=ϕϕϕϕ,)0(00D is a bounded linear operator from B into E and for each x : (−∞, T ] → E, T > 0, and t ∈ [0,T ], xt represents, as usual, the mapping from (−∞, 0] into E deﬁned by]0,(),()(-∞∈+=θθθt x xtF is an E-valued nonlinear continuous mapping on B ⨯ℜ+.The problem of controllability of linear and nonlinear systems repr esented by ODE in ﬁnit dimensional space was extensively studied. Many authors extended the controllability concept to inﬁnite dimensional systems in Banach space with unbounded operators. Up to now, there are a lot of works on this topic, see, for example, [4, 7, 10, 21]. There are many systems that can be written as abstract neutral evolution equations with inﬁnite delay to study [23]. In recent years, the theory of neutral functional di ﬀerential equations with inﬁnite delay in inﬁnitedimension was deve loped and it is still a ﬁeld of research (see, for instance, [2, 9, 14, 15] and the references therein). Meanwhile, the controllability problem of such systems was also discussed by many mathematicians, see, for example, [5, 8]. The objective of this article is to discuss the controllability for Eq. (1), where the linear part is supposed to be non-densely deﬁned but satisﬁes the resolvent estimates of the Hille-Yosida theorem. We shall assume conditions that assure global existence and give the su ﬃcient conditions for controllability of some partial neutral functional di ﬀerential equations with inﬁnite delay. The results are obtained using the integrated semigroups theory and Banach ﬁxed point theorem. Besides, we make use of the notion of integral solution and we do not use the analytic semigroups theory.Treating equations with inﬁnite delay such as Eq. (1), we need to introduce the phase space B. To avoid repetitions and understand the interesting properties of the phase space, suppose that ).,(B B is a (semi)normed abstract linear space of functions mapping (−∞, 0] into E, and satisﬁes the following fundamental axioms that were ﬁrst introduced in [13] and widely discussedin [16].(A)There exist a positive constant H and functions K(.), M(.):++ℜ→ℜ,with K continuous and M locally bounded, such that, for any ℜ∈σand 0>a ,if x : (−∞, σ + a] → E, B x ∈σ and (.)x is continuous on [σ, σ+a], then, for every t in [σ, σ+a], the following conditions hold:(i) B xt ∈, (ii) Bt x H t x ≤)(,which is equivalent toB H ϕϕ≤)0(or every B ∈ϕ(iii) Bσσσσx t M s x t K xtts B)()(sup )(-+-≤≤≤(A) For the function (.)x in (A), t → xt is a B -valued continuous function for t in [σ, σ + a]. (B) The space B is complete.Throughout this article, we also assume that the operator A satisﬁes the Hille -Yosida condition :(H1) There exist and ℜ∈ω，such that )(),(A ρω⊂+∞ and {}M N n A I n n ≤≥∈---ωλλωλ,:)()(sup （2） Let A0 be the part of operator A in )(A D deﬁned by{}⎩⎨⎧∈=∈∈=)(,,)(:)()(000A D x for Ax x A A D Ax A D x A D It is well known that )()(0A D A D =and the operator 0A generates a strongly continuoussemigroup ))((00≥t t T on )(A D .Recall that [19] for all )(A D x ∈ and 0≥t ,one has )()(000A D xds s T f t∈ andx t T x sds s T A t )(0)(00=+⎪⎭⎫ ⎝⎛⎰. We also recall that 00))((≥t t T coincides on )(A D with the derivative of the locally Lipschitz integrated semigroup 0))((≥t t S generated by A on E, which is, according to [3, 17, 18],a family of bounded linear operators on E, that satisﬁes(i) S(0) = 0, (ii) for any y ∈ E, t → S(t)y is strongly continuous with values in E,(iii)⎰-+=sdr r s r t S t S s S 0))()(()()(for all t, s ≥ 0, and for any τ > 0 there exists aconstant l(τ) > 0, such thats t l s S t S -≤-)()()(τ or all t, s ∈ [0, τ] .The C0-semigroup 0))((≥'t t S is exponentially bounded, that is, there exist two constantsM and ω,such that t e M t S ω≤')( for all t ≥ 0.Notice that the controllability of a class of non-de nsely deﬁned functional di ﬀerential equations was studied in [12] in the ﬁnite delay case.、2 Main ResultsWe start with introducing the following deﬁnition.Deﬁnition 1 Let T > 0 and ϕ ∈ B. We consider the following deﬁnition.We say that a function x := x(., ϕ) : (−∞, T ) → E, 0 < T ≤ +∞, is an integral solution of Eq. (1) if(i) x is continuous on [0, T ) ,(ii) ⎰∈tA D Dxsds 0)( for t ∈ [0, T ) ,(iii) ⎰⎰+++=ts tt ds x s F s Cu Dxsds A D Dx 0),()(ϕfor t ∈ [0, T ) ,(iv))()(t t x ϕ= for all t ∈ (−∞, 0].We deduce from [1] and [22] that integral solutions of Eq. (1) are given for ϕ ∈ B, such that )(A D D ∈ϕ by the following system⎪⎩⎪⎨⎧-∞∈=∈+-'+'=⎰+∞→],0,(),()(),,0[,)),()(()(lim )(0t t t x t t ds x s F s Cu B s t S D t S Dxt ts ϕλϕλ 、 (3)Where 1)(--=A I B λλλ.To obtain global existence and uniqueness, we supposed as in [1] that (H2) 1)0(0<D K .(H3) E F →B ⨯+∞],0[:is continuous and there exists 0β> 0, such that B -≤-21021),(),(ϕϕβϕϕt F t F for ϕ1, ϕ2 ∈ B and t ≥ 0. (4)Using Theorem 7 in [1], we obtain the following result.Theorem 1 Assume that (H1), (H2), and (H3) hold. Let ϕ ∈ B such that D ϕ ∈ D(A). Then, there exists a unique integral solution x(., ϕ) of Eq. (1), deﬁned on (−∞,+∞) .Deﬁnition 2 Under the above conditions, Eq. (1) is said to be controllable on the interval J = [0, δ], δ > 0, if for every initial function ϕ ∈ B with D ϕ ∈ D(A) and for any e1 ∈ D(A), there exists a control u ∈ L2(J,U), such that the solution x(.) of Eq. (1) satisﬁes 1)(e x =δ.Theorem 2 Suppose that(H1), (H2), and (H3) hold. Let x(.) be the integral solution of Eq. (1) on (−∞, δ) , δ > 0, and assume that (see [20]) the linear operator W from U into D(A) deﬁned byds s Cu B s S Wu )()(limλδλδ⎰-'=+∞→，（5）nduces an invertible operator W ~on KerW U J L /),(2，such that there exist positive constants1N and 2N satisfying 1N C ≤and 21~N W ≤-，then, Eq. (1) is controllable on J providedthat1))(2221000<++δδωδωδβδβK e M N N e M D ，（6）Where)(max :0t K K t δδ≤≤=.Proof Following [1], when the integral solution x(.) of Eq. (1) exists on (−∞, δ) , δ > 0, it is given for all t ∈ [0, δ] byds s Cu s t S dt d ds x s F s t S dt d D t S x D t x tt s t ⎰⎰-+-+'+=000)()(),()()()(ϕOrdsx s B s t S D t S x D t x tst ⎰-'+'+=+∞→00),()(lim)()(λλϕds s Cu B s t S t⎰-'++∞→0)()(limλλThen, an arbitrary integral solution x(.) of Eq. (1) on (−∞, δ) , δ > 0, satisﬁes x(δ) = e1 if and only ifdss Cu B s t S ds x s F s S d d D S x D e ts ⎰⎰-'+-+'+=+∞→001)()(lim),()()(λλδδδδϕδThis implies that, by use of (5), it su ﬃces to take, for all t ∈ J,{})()()(lim~)(01t ds s Cu B s t S W t u t⎰-'=+∞→-λλ{})(),()(lim )(~011t ds x s B s t S D S x D e W ts⎰-'-'--=+∞→-λλϕδδin order to have x(δ) = e1. Hence, we must take the control as above, and consequently, the proof is reduced to the existence of the integral solution given for all t ∈ [0, δ] by⎰-+'+=ts t ds z s F s t S dtd D t S z D t Pz 00),()()(:))((ϕ {ϕδδδD S z D z W C s t S dt d t )()(~)(001'---=⎰- ds s d z F B S )}(),()(limτττδτλδλ⎰-'-+∞→Without loss of generality, suppose thatω ≥ 0. Using similar arguments as in [1], we can seehat, for every1z ,)(2ϕδZ z ∈and t ∈ [0, δ] ,∞-+≤-210021)())(())((z z K e M D t Pz t Pz δδωβAs K is continuous and1)0(0<K D ,we can choose δ > 0 small enough, such that1)2221000<++δδωδωδββK e M N N e M D .Then, P is a strict contraction in )(ϕδZ ,and the ﬁxed point of P gives the unique integral olution x(., ϕ) on (−∞, δ] that veriﬁes x(δ) = e1.Remark 1 Suppose that all linear operators W from U into D(A) deﬁned byds s Cu B s b S Wu )()(limλδλ⎰-'=+∞→0 ≤ a < b ≤ T, T > 0, induce invertible operators W ~ on KerW U b a L /)],,([2,such that thereexist positive constants N1 and N2 satisfying 1N C ≤ and21~N W ≤-,taking NT =δ,N large enough and following [1]. A similar argument as the above proof can be used inductively in 11],)1(,[-≤≤+N n n n δδ,to see that Eq. (1) is controllable on [0, T ] for all T > 0.Acknowledgements The authors would like to thank Prof. Khalil Ezzinbi and Prof. Pierre Magal for the fruitful discussions.References[1] Adimy M, Bouzahir H, Ezzinbi K. Existence and stability for some partial neutral functional di ﬀerenti al equations with inﬁnite delay. J Math Anal Appl, 2004, 294: 438–461[2] Adimy M, Ezzinbi K. A class of linear partial neutral functional diﬀerential equations withnondense domain. J Dif Eq, 1998, 147: 285–332[3] Arendt W. Resolvent positive operators and integrated semigroups. Proc London Math Soc,1987, 54(3):321–349[4] Atmania R, Mazouzi S. Controllability of semilinear integrodiﬀerential equations withnonlocal conditions. Electronic J of Diﬀ Eq, 2005, 2005: 1–9[5] Balachandran K, Anandhi E R. Controllability of neutral integrodiﬀerential inﬁnite delaysystems in Banach spaces. Taiwanese J Math, 2004, 8: 689–702[6] Balasubramaniam P, Ntouyas S K. Controllability for neutral stochastic functional diﬀerentialinclusionswith inﬁnite delay in abst ract space. J Math Anal Appl, 2006, 324(1): 161–176、[7] Balachandran K, Balasubramaniam P, Dauer J P. Local null controllability of nonlinearfunctional diﬀer-ential systems in Banach space. J Optim Theory Appl, 1996, 88: 61–75 [8] Balasubramaniam P, Loganathan C. Controllability of functional diﬀerential equations withunboundeddelay in Banach space. J Indian Math Soc, 2001, 68: 191–203[9] Bouzahir H. On neutral functional diﬀerential equations. Fixed Point Theory, 2005, 5: 11–21 The study of differential equations is one part of mathematics that, perhaps more than any other, has been directly inspired by mechanics, astronomy, and mathematical physics. Its history began in the 17th century when Newton, Leibniz, and the Bernoullis solved some simple differential equation arising from problems in geometry and mechanics. There early discoveries, beginning about 1690, gradually led to the development of a lot of “special tricks” for solving certain special kinds of differential equations. Although these special tricks are applicable in mechanics and geometry, so their study is of practical importance.微分方程牛顿和莱布尼茨，完全相互独立，主要负责开发积分学思想的地步，迄今无法解决的问题可以解决更多或更少的常规方法。

高等数学名词(中英文对照)

高等数学名词(中英文)第一章函数与极限Chapter1 Function and Limit 集合set 元素element子集subset空集empty set并集union交集intersection 差集difference of set基本集basic set 补集complement set直积direct product笛卡儿积Cartesian product开区间open interval 闭区间closed interval 半开区间half open interval 有限区间finite interval区间的长度length of an interval无限区间infinite interval邻域neighborhood邻域的中心centre of a neighborhood 邻域的半径radius of a neighborhood 左邻域left neighborhood右邻域right neighborhood映射mappingX 到Y 的映射mapping of X onto Y 满射surjection单射injection一一映射one-to-one mapping双射bijection算子operator变化transformation函数function逆映射inverse mapping复合映射composite mapping自变量independent variable 因变量dependent variable定义域domain函数值value of function函数关系function relation值域range自然定义域natural domain单值函数single valued function多值函数multiple valued function单值分支one-valued branch函数图形graph of a function绝对值函数absolute value符号函数sigh function整数部分integral part阶梯曲线step curve当且仅当if and only if(iff)分段函数piecewise function上界upper bound下界lower bound有界boundedness无界unbounded函数的单调性monotonicity of a function 单调增加的increasing单调减少的decreasing单调函数monotone function函数的奇偶性parity(odevity) of a function 对称symmetry偶函数even function奇函数odd function函数的周期性periodicity of a function周期period反函数inverse function直接函数direct function复合函数composite function中间变量intermediate variable函数的运算operation of function基本初等函数basic elementary function初等函数elementary function幂函数power function指数函数exponential function对数函数logarithmic function三角函数trigonometric function反三角函数inverse trigonometric function 常数函数constant function双曲函数hyperbolic function双曲正弦hyperbolic sine双曲余弦hyperbolic cosine双曲正切hyperbolic tangent反双曲正弦inverse hyperbolic sine反双曲余弦inverse hyperbolic cosine反双曲正切inverse hyperbolic tangent极限limit数列sequence of number收敛convergence收敛于converge to发散divergent极限的唯一性uniqueness of limits收敛数列的有界性boundedness of a convergent sequence子列subsequence函数的极限limits of functions函数当x 趋于x0 时的极限limit of functions as x approaches x0左极限left limit右极限right limit单侧极限one-sided limits水平渐近线horizontal asymptote无穷小infinitesimal无穷大infinity铅直渐近线vertical asymptote夹逼准则squeeze rule单调数列monotonic sequence高阶无穷小infinitesimal of higher order 低阶无穷小infinitesimal of lower order 同阶无穷小infinitesimal of the same order 等阶无穷小equivalent infinitesimal 函数的连续性continuity of a function增量increment函数在x0 连续the function is continuous at x0左连续left continuous右连续right continuous区间上的连续函数continuous function函数在该区间上连续function is continuous on an interval不连续点discontinuity point第一类间断点discontinuity point of the first kind第二类间断点discontinuity point of the second kind初等函数的连续性continuity of the elementary functions定义区间defined interval最大值global maximum value (absolute maximum)最小值global minimum value (absolute minimum)零点定理the zero-point theorem介值定理intermediate value theorem第二章导数与微分Chapter2 Derivative and Differential速度velocity匀速运动uniform motion平均速度average velocity瞬时速度instantaneous velocity圆的切线tangent line of a circle切线tangent line切线的斜率slope of the tangent line位置函数position function导数derivative可导derivable函数的变化率问题problem of the change rate of a function导函数derived function左导数left-hand derivative右导数right-hand derivative单侧导数one-sided derivatives在闭区间[a, b] 上可导is derivable on the closed interval [a,b]切线方程tangent equation角速度angular velocity成本函数cost function边际成本marginal cost链式法则chain rule隐函数implicit function显函数explicit function二阶函数second derivative三阶导数third derivative高阶导数nth derivative莱布尼茨公式Leibniz formula对数求导法log- derivative参数方程parametric equation相关变化率correlative change rata微分differential可微的differentiable函数的微分differential of function自变量的微分differential of independent variable微商differential quotient间接测量误差indirect measurement error 绝对误差absolute error相对误差relative error第三章微分中值定理与导数的应用Chapter3 Mean Value Theorem ofDifferentials and the Application ofDerivatives罗马定理Rolle’s theorem费马引理Fermat’s lemma拉格朗日中值定理Lagrange’s mean value theorem驻点stationary point稳定点stable point 临界点critical point辅助函数auxiliary function拉格朗日中值公式Lagrange’s mean value formula柯西中值定理Cauchy’s mean value theorem 洛必达法则L’Hospital’s Rule0/0 型不定式indeterminate form of type 0/0 不定式indeterminate form泰勒中值定理Taylor’s mean value theorem 泰勒公式Taylor formula余项remainder term拉格朗日余项Lagrange remainder term麦克劳林公式Maclaurin’s formula佩亚诺公式Peano remainder term凹凸性concavity凹向上的concave upward, concave up凹向下的，向上凸的concave downward’ concave down拐点inflection point函数的极值extremum of function极大值local(relative) maximum最大值global(absolute) maximum极小值local(relative) minimum最小值global(absolute) minimum目标函数objective function曲率curvature弧微分arc differential平均曲率average curvature曲率园circle of curvature曲率中心center of curvature曲率半径radius of curvature渐屈线evolute渐伸线involute根的隔离isolation of root隔离区间isolation interval切线法tangent line method第四章不定积分Chapter4 Indefinite Integrals原函数primitive function(anti-derivative)积分号sign of integration被积函数integrand积分变量integral variable积分曲线integral curve积分表table of integrals换元积分法integration by substitution分部积分法integration by parts分部积分公式formula of integration by parts 有理函数rational function真分式proper fraction假分式improper fraction第五章定积分Chapter5 Definite Integrals曲边梯形trapezoid with曲边curve edge窄矩形narrow rectangle曲边梯形的面积area of trapezoid with curved edge积分下限lower limit of integral积分上限upper limit of integral积分区间integral interval分割partition积分和integral sum可积integrable矩形法rectangle method积分中值定理mean value theorem of integrals函数在区间上的平均值average value of a function on an intervals牛顿－莱布尼茨公式Newton-Leibniz formula微积分基本公式fundamental formula of calculus换元公式formula for integration by substitution递推公式recurrence formula反常积分improper integral 反常积分发散the improper integral is divergent反常积分收敛the improper integral is convergent无穷限的反常积分improper integral on an infinite interval无界函数的反常积分improper integral of unbounded functions绝对收敛absolutely convergent第六章定积分的应用Chapter6 Applications of the Definite Integrals元素法the element method面积元素element of area平面图形的面积area of a plane figure直角坐标又称“笛卡儿坐标(Cartesian coordinates)”极坐标polar coordinates抛物线parabola椭圆ellipse旋转体的面积volume of a solid of rotation 旋转椭球体ellipsoid of revolution, ellipsoid of rotation曲线的弧长arc length of a curve可求长的rectifiable光滑smooth功work水压力water pressure引力gravitation变力variable force第七章空间解析几何与向量代数Chapter7 Space Analytic Geometry and Vector Algebra向量vector自由向量free vector单位向量unit vector零向量zero vector相等equal平行parallel向量的线性运算linear poeration of vector三角法则triangle rule 平行四边形法则parallelogram rule 交换律commutative law结合律associative law负向量negative vector差difference分配律distributive law空间直角坐标系space rectangular coordinates坐标面coordinate plane卦限octant向量的模modulus of vector向量a 与b 的夹角angle between vector a and b方向余弦direction cosine方向角direction angle向量在轴上的投影projection of a vector onto an axis数量积，外积，叉积scalar product,dot product,inner product曲面方程equation for a surface球面sphere旋转曲面surface of revolution母线generating line轴axis 圆锥面cone 顶点vertex旋转单叶双曲面revolution hyperboloids of one sheet旋转双叶双曲面revolution hyperboloids of two sheets柱面cylindrical surface ,cylinder圆柱面cylindrical surface准线directrix抛物柱面parabolic cylinder二次曲面quadric surface 椭圆锥面dlliptic cone椭球面ellipsoid单叶双曲面hyperboloid of one sheet 双叶双曲面hyperboloid of two sheets 旋转椭球面ellipsoid of revolution 椭圆抛物面elliptic paraboloid 旋转抛物面paraboloid of revolution 双曲抛物面hyperbolic paraboloid 马鞍面saddle surface椭圆柱面elliptic cylinder 双曲柱面hyperbolic cylinder 抛物柱面parabolic cylinder 空间曲线spacecurve空间曲线的一般方程general form equations of a space curve空间曲线的参数方程parametric equations of a space curve螺转线spiral 螺矩pitch投影柱面projecting cylinder投影projection平面的点法式方程pointnorm form eqyation of a plane法向量normal vector平面的一般方程general form equation of a plane两平面的夹角angle between two planes点到平面的距离distance from a point to a plane空间直线的一般方程general equation of a line in space方向向量direction vector直线的点向式方程pointdirection form equations of a line方向数direction number直线的参数方程parametric equations of a line两直线的夹角angle between two lines垂直perpendicular直线与平面的夹角angle between a line and a planes平面束pencil of planes平面束的方程equation of a pencil of planes 行列式determinant系数行列式coefficient determinant第八章多元函数微分法及其应用Chapter8 Differentiation of Functions of Several Variables and Its Application一元函数function of one variable多元函数function of several variables内点interior point外点exterior point边界点frontier point,boundary point聚点point of accumulation开集openset闭集closed set 连通集connected set 开区域open region 闭区域closed region 有界集bounded set 无界集unbounded setn 维空间n-dimentional space二重极限double limit多元函数的连续性continuity of function of seveal连续函数continuous function不连续点discontinuity point 一致连续uniformly continuous 偏导数partial derivative对自变量x 的偏导数partial derivative with respect to independent variable x高阶偏导数partial derivative of higher order 二阶偏导数second order partial derivative 混合偏导数hybrid partial derivative 全微分total differential偏增量oartial increment偏微分partial differential 全增量total increment 可微分differentiable 必要条件necessary condition 充分条件sufficient condition叠加原理superpostition principle全导数total derivative中间变量intermediate variable 隐函数存在定理theorem of the existence of implicit function曲线的切向量tangent vector of a curve法平面normal plane向量方程vector equation 向量值函数vector-valued function 切平面tangent plane法线normal line方向导数directional derivative梯度gradient 数量场scalar field 梯度场gradient field 向量场vector field 势场potential field引力场gravitational field引力势gravitational potential曲面在一点的切平面tangent plane to a surface at a point曲线在一点的法线normal line to a surface at a point无条件极值unconditional extreme values 条件极值conditional extreme values 拉格朗日乘数法Lagrange multiplier method 拉格朗日乘子Lagrange multiplier 经验公式empirical formula最小二乘法method of least squares均方误差mean square error第九章重积分Chapter9 Multiple Integrals二重积分double integral可加性additivity累次积分iterated integral体积元素volume element三重积分triple integral直角坐标系中的体积元素volume element in rectangular coordinate system柱面坐标cylindrical coordinates柱面坐标系中的体积元素volume element in cylindrical coordinate system球面坐标spherical coordinates球面坐标系中的体积元素volume element in spherical coordinate system反常二重积分improper double integral曲面的面积area of a surface质心centre of mass静矩static moment密度density形心centroid转动惯量moment of inertia参变量parametric variable第十章曲线积分与曲面积分Chapter10 Line (Curve) Integrals andSurface Integrals对弧长的曲线积分line integrals with respect to arc hength第一类曲线积分line integrals of the first type对坐标的曲线积分line integrals with respect to x,y,and z第二类曲线积分line integrals of the second type有向曲线弧directed arc单连通区域simple connected region 复连通区域complex connected region 格林公式Green formula第一类曲面积分surface integrals of the first type对面的曲面积分surface integrals with respect to area 有向曲面directed surface对坐标的曲面积分surface integrals with respect to coordinate elements第二类曲面积分surface integrals of the second type有向曲面元element of directed surface高斯公式gauss formula 拉普拉斯算子Laplace operator 格林第一公式Green’s first formula 通量flux散度divergence 斯托克斯公式Stokes formula 环流量circulation旋度rotation,curl第十一章无穷级数Chapter11 Infinite Series一般项general term 部分和partial sum 余项remainder term等比级数geometric series几何级数geometric series公比common ratio 调和级数harmonic series柯西收敛准则Cauchy convergence criteria, Cauchy criteria for convergence 正项级数series of positive terms 达朗贝尔判别法D’Alembert test柯西判别法Cauchy test 交错级数alternating series 绝对收敛absolutely convergent条件收敛conditionally convergent柯西乘积Cauchy product 函数项级数series of functions 发散点point of divergence 收敛点pointof convergence 收敛域convergence domain 和函数sumfunction幂级数power series幂级数的系数coeffcients of power series阿贝尔定理Abel Theorem 收敛半径radius of convergence 收敛区间interval of convergence 泰勒级数Taylor series 麦克劳林级数Maclaurin series 二项展开式binomial expansion 近似计算approximate calculation舍入误差round-off error,rounding error 欧拉公式Euler’s formula 魏尔斯特拉丝判别法Weierstrass test 三角级数trigonometric series振幅amplitude角频率angular frequency初相initial phase矩形波square wave 谐波分析harmonic analysis 直流分量direct component 基波fundamental wave 二次谐波second harmonic三角函数系trigonometric function system 傅立叶系数Fourier coefficient 傅立叶级数Forrier series周期延拓periodic prolongation正弦级数sine series 余弦级数cosine series 奇延拓oddprolongation 偶延拓evenprolongation傅立叶级数的复数形式complex form of Fourier series第十二章微分方程Chapter12 Differential Equation 解微分方程solve a differential equation 常微分方程ordinary differential equation 偏微分方程partial differential equation,PDE 微分方程的阶order of a differential equation 微分方程的解solution of a differential equation 微分方程的通解general solution of a differential equation初始条件initial condition微分方程的特解particular solution of a differential equation初值问题initial value problem微分方程的积分曲线integral curve of a differential equation可分离变量的微分方程variable separable differential equation隐式解implicit solution 隐式通解inplicit general solution 衰变系数decay coefficient衰变decay齐次方程homogeneous equation 一阶线性方程linear differential equation of first order 非齐次non-homogeneous齐次线性方程homogeneous linear equation 非齐次线性方程non-homogeneous linear equation常数变易法method of variation of constant 暂态电流transient state current 稳态电流steady state current 伯努利方程Bernoulli equation 全微分方程total differential equation 积分因子integrating factor高阶微分方程differential equation of higher order悬链线catenary高阶线性微分方程linear differential equation of higher order 自由振动的微分方程differential equation of free vibration强迫振动的微分方程differential equation of forced oscillation串联电路的振荡方程oscillation equation of series circuit二阶线性微分方程second order lineardifferential equation线性相关linearly dependence线性无关linearly independence 二阶常系数齐次线性微分方程second order homogeneous linear differential equation with constant coefficient二阶变系数齐次线性微分方程second order homogeneous linear differential equation with variable coefficient特征方程characteristic equation无阻尼自由振动的微分方程differential equation of free vibration with zero damping 固有频率natural frequency 简谐振动simple harmonic oscillation,simple harmonic vibration微分算子differential operator待定系数法method of undetermined coefficient共振现象resonance phenomenon欧拉方程Euler equation 幂级数解法power series solution 数值解法numerial solution 勒让德方程Legendre equation微分方程组system of differential equations 常系数线性微分方程组system of linear differential equations with constant coefficie。

数学专业英语课文翻译2-9,2-10

No matter what the students’ field of major interest is , knowledge of the rudiments of matrices is likely to broaden the range of literature that he can read with understanding .
2.9 微分方程简介 Introduction to Differential Equations
New Words & Expressions:
approximate evaluation 近似估计 initial 初始的
disintegrate 解体，衰变
differentiable 可微的 exponential 指数的数
Or a radioactive substance may be disintegrating at a known rate and we may be required to determine the amount of material present after a given time.
又如，某种放射性物质可能正在以已知的速度进行衰变，需要我们确定在给定的时间后遗留物质的总量。
※In examples like these, we are trying to determine an unknown function from prescribed information expressed in the form of an equation involving at least one of the derivatives of the unknown function . 在类似的例子中，我们力求由方程的形式表述的信息来确定未知函数，而这种方程至少包含了未知函数的一个导数。

数学专业英语2-2

点没有长度，宽度和厚度，但是标记了一个位置。我们熟悉铅笔尖，针尖这样的表达。我们可以用一个小圆点来表示一个点，在它旁边用打印体大写字母来命名，如图2-2-1中的点A。
The line is named by labeling two points on it with capital letters or one small letter near it. The straight line extends infinitely far in two directions and has no ends. The part of the line between two points on the line is termed a line segment.
我们都熟悉直线，但是很难定义直线。可能可以用移动一支铅笔或者钢笔横穿一张纸留下的痕迹来描述直线。
直线可以被看做是一维的，即只有长度。尽管我们画一条直线的时候会赋予它宽度和厚度，但是当考虑直线的时候，只考虑痕迹的长度。
A point has no length, no width, and no thickness, but marks a position. We are familiar with such expressions as pencil point and needle point. We represent a point by a small dot and name it by a capital letter printed beside it, as “point A” in Fig. 2-2-1.
有三种线：直线，折线和曲线。曲线是指其中没有任何部分是直的。折线是由连起来的直线段构成，如图2-2-3中的ABCDE.

数学专业英语课后翻译

2.1 数学、方程与比例（1）数学来源于人类的社会实践，包括工农业的劳动，商业、军事和科学技术研究等活动。

Mathematics comes from man’s social practice, for example, industrial and agricultural production, commercial activities, military operations and scientific and technological researches.（2）如果没有运用数学，任何一个科学技术分支都不可能正常地发展。

No modern scientific and technological branches could be regularly developed without the application of mathematics.（3）符号在数学中起着非常重要的作用，它常用于表示概念和命题。

Notations are a special and powerful tool of mathematics and are used to express conceptions and propositions very often.（4）17 世纪之前，人们局限于初等数学，即几何、三角和代数，那时只考虑常数。

Before 17th century, man confined himself to the elementary mathematics, i. e. , geometry, trigonometry and algebra, in which only the constants were considered.（5）方程与算数的等式不同在于它含有可以参加运算的未知量。

Equation is different from arithmetic identity in that it contains unknown quantity which can join operations.（6）方程又称为条件等式，因为其中的未知量通常只允许取某些特定的值。

数学专业英语翻译

第一段翻译‎(2)：what is the exact‎ value‎o f the numbe‎r pai?a mathe‎matic‎i an made an exper‎i ment‎in order‎to find his own estim‎a tion‎of the numbe‎r pai.in his exper‎i ment‎,he used an old bicyc‎l e wheel‎of diame‎t er 63.7cm.he marke‎d the point‎on the tire where‎the wheel‎was touch‎i ng the groun‎d and he rolle‎d the wheel‎strai‎g ht ahead‎by turni‎n g it 20 times‎.next,he measu‎r ed the dista‎n ce trave‎l ed by the wheel‎,which‎was 39.69 meter‎s.he divid‎e d the numbe‎r3969 by 20*63.7 and obtai‎n ed 3.11538‎4615 as an appro‎x imat‎i on of the numbe‎r pai.of cours‎e,this was just his estim‎a te of the numbe‎r pai and he was aware‎that it was not very accur‎a te.数π的精确‎值是什么？一位数学家‎做了实验以‎便找到他自‎己对数π的‎估计。

在试验中，他用了一直‎径63.1厘米的旧‎自行车轮。

他在车轮接‎触地面的轮‎胎上做了标‎记，而且将车轮‎向前转动2‎0次。

接下来，他测量了车‎轮经过的距‎离，是39.69米。

他用396‎9除20*63.7得到了数‎π的近似值‎3.11538‎4615。

数学专业英语课文翻译2(吴炯圻)

7A在日常使用的英文单词"序列"和' '系列"是同义词，和他们用来建议一系列的事情或按某种顺序排列的事件。

在数学中，这句话有特别技术的意义。

"序列"一词被受雇如在共同使用这一术语，传达的理念的一套东西排列顺序，但"系列"一词用于稍有不同的意义。

概念在本节中，将讨论序列和系列将定义第11 节。

如果为每个正整数n 有关联的真实或复数a，那时有序的集据说是定义一个无限的序列。

这里最重要的是每个成员集的已标记的整数，使我们可以发言的第一届、第二个任期，以及，一般的第n 个词。

每个学期了继任者，因此，没有任何"最后"一词。

如果我们给一些规则或第n 个词描述的公式，可以构造序列的最常见的例子。

因此，例如，公式= 1 定义的序列的第五个任期是1.有时两个或多个公式可受雇作为，例如，a=1.the 第一次在这种情况下被1 的一些术语。

另一种常见方法定义一系列是一套的说明解释了如何在一个给定的开始后进行的。

因此，我们可能= 1。

此特定的规则被称为递归公式，它定义了著名的序列，其条款被称为斐波那契数。

第一次的几个术语are1。

最重要的事情是序列的序列的这样f(n) 的每个n=1.In 事实的第n 个燕鸥是序列的序列的正整数上定义一些函数 f 的任何序列，这可能是序列的序列的最方便的方法，国家技术定义。

定义。

其域是所有积极integers1 的一组函数f 称为一个无限的序列。

函数值f(n) 调用序列的第n 个词。

通过按顺序，因此编写条款通常显示的功能（即，函数值的集合）的范围：f (2)。

为简便起见，{f(n)} 符号用于指示第n 个任期是f(n) 的序列。

由使用下标，很多时候表示，n 的依赖和我们写，或类似的而不是f (n0。

除非另外指定，否则所有的序列，在这一章中假定有真实的或复杂的条款。

7B我们担心在这里主要的问题在于决定是否条款f(n) 倾向于有限的n 无限增加。

数学专业英语课文翻译(比较全)

1－A What is mathematics
Mathematics comes from man’s social practice, for example, industrial and agricultural production, commercial activities, military operations and scientific and technological researches. And in turn, mathematics serves the practice and plays a great role in all fields. No modern scientific and technological branches could be regularly developed without the application of mathematics. 数学来源于人类的社会实践，比如工农业生产，商业活动，军事行动和科学技术研究。反过来，数学服务于实践，并在各个领域中起着非常重要的作用。没有应用数学，任何一个现在的科技的分支都不能正常发展。From the early need of man came the concepts of numbers and forms. Then, geometry developed out of problems of measuring land , and trigonometry came from problems of surveying . To deal with some more complex practical problems, man established and then solved equation with unknown numbers ,thus algebra occurred. Before 17th century, man confined himself to the elementary mathematics, i.e. , geometry, trigonometry and algebra, in which only the constants are considered. 很早的时候，人类的需要产生了数和形式的概念，接着，测量土地的需要形成了几何，出于测量的需要产生了三角几何，为了处理更复杂的实际问题，人类建立和解决了带未知参数的方程，从而产生了代数学，17世纪前，人类局限于只考虑常数的初等数学，即几何，三角几何和代数。The rapid development of industry in 17th century promoted the progress of economics and technology and required dealing with variable quantities. The leap from constants to variable quantities brought about two new branches of mathematics----analytic geometry and calculus, which belong to the higher mathematics. Now there are many branches in higher mathematics, among which are mathematical analysis, higher algebra, differential equations, function theory and so on. 17世纪工业的快速发展推动了经济技术的进步，从而遇到需要处理变量的问题，从常数带变量的跳跃产生了两个新的数学分支-----解析几何和微积分，他们都属于高等数学，现在高等数学里面有很多分支，其中有数学分析，高等代数，微分方程，函数论等。Mathematicians study conceptions and propositions, Axioms, postulates, definitions and theorems are all propositions. Notations are a special and powerful tool of mathematics and are used to express conceptions and propositions very often. Formulas ,figures and charts are full of different symbols. Some of the best known symbols of mathematics are the Arabic numerals 1,2,3,4,5,6,7,8,9,0 and the signs of addition, subtraction , multiplication, division and equality. 数学家研究的是概念和命题，公理，公设，定义和定理都是命题。符号是数学中一个特殊而有用的工具，常用于表达概念和命题。公式，图表都是不同的符号……..The conclusions in mathematics are obtained mainly by logical deductions and computation. For a long period of the history of mathematics, the centric place of mathematics methods was occupied by the logical deductions. Now , since electronic computers are developed promptly and used widely, the role of computation becomes more and more important. In our times, computation is not only used to deal with a lot of information and data, but also to carry out some work that merely could be done earlier by logical deductions, for example, the proof of most of geometrical theorems. 数学结论主要由逻辑推理和计算得到，在数学发展历史的很长时间内，逻辑推理一直占据着数学方法的中心地位，现在，由于电子计算机的迅速发展和广泛使用，计算机的地位越来越重要，现在计算机不仅用于处理大量的信息和数据，还可以完成一些之前只能由逻辑推理来做的工作，例如，大多数几何定理的证明。 Biblioteka 1－B Equation

高等数学名词（中英文）

高等数学名词（中英文）高等数学名词(中英文)第一章函数与极限Chapter1 Function and Limit 集合set元素element子集subset空集empty set并集union交集intersection差集difference of set基本集basic set补集complement set直积direct product笛卡儿积Cartesian product开区间open interval闭区间closed interval半开区间half open interval有限区间finite interval区间的长度length of an interval无限区间infinite interval领域neighborhood领域的中心centre of a neighborhood 领域的半径radius of a neighborhood 左领域left neighborhood右领域right neighborhood映射mappingX到Y的映射mapping of X ontoY 满射surjection单射injection一一映射one-to-one mapping双射bijection算子operator变化transformation函数function逆映射inverse mapping复合映射composite mapping自变量independent variable 因变量dependent variable定义域domain函数值value of function函数关系function relation值域range自然定义域natural domain单值函数single valued function多值函数multiple valued function单值分支one-valued branch函数图形graph of a function绝对值函数absolute value符号函数sigh function整数部分integral part阶梯曲线step curve当且仅当if and only if(iff)分段函数piecewise function上界upper bound下界lower bound有界boundedness无界unbounded函数的单调性monotonicity of a function 单调增加的increasing单调减少的decreasing单调函数monotone function函数的奇偶性parity(odevity) of a function 对称symmetry偶函数even function奇函数odd function函数的周期性periodicity of a function周期period反函数inverse function直接函数direct function复合函数composite function中间变量intermediate variable函数的运算operation of function基本初等函数basic elementary function 初等函数elementary function幂函数power function指数函数exponential function对数函数logarithmic function三角函数trigonometric function反三角函数inverse trigonometric function 常数函数constant function双曲函数hyperbolic function双曲正弦hyperbolic sine双曲余弦hyperbolic cosine双曲正切hyperbolic tangent反双曲正弦inverse hyperbolic sine反双曲余弦inverse hyperbolic cosine反双曲正切inverse hyperbolic tangent极限limit数列sequence of number收敛convergence收敛于a converge to a发散divergent极限的唯一性uniqueness of limits收敛数列的有界性boundedness of a convergent sequence子列subsequence函数的极限limits of functions函数当x趋于x0时的极限limit of functions as x approaches x0左极限left limit右极限right limit单侧极限one-sided limits水平渐近线horizontal asymptote无穷小infinitesimal无穷大infinity铅直渐近线vertical asymptote夹逼准则squeeze rule单调数列monotonic sequence高阶无穷小infinitesimal of higher order低阶无穷小infinitesimal of lower order同阶无穷小infinitesimal of the same order等阶无穷小equivalent infinitesimal 函数的连续性continuity of a function增量increment函数在x0连续the function is continuous at x0左连续left continuous右连续right continuous区间上的连续函数continuous function函数在该区间上连续function is continuous on an interval不连续点discontinuity point第一类间断点discontinuity point of the first kind第二类间断点discontinuity point of the second kind初等函数的连续性continuity of the elementary functions定义区间defined interval最大值global maximum value (absolute maximum)最小值global minimum value (absolute minimum)零点定理the zero point theorem介值定理intermediate value theorem第二章导数与微分Chapter2 Derivative and Differential速度velocity匀速运动uniform motion平均速度average velocity瞬时速度instantaneous velocity圆的切线tangent line of a circle切线tangent line切线的斜率slope of the tangent line位置函数position function导数derivative可导derivable函数的变化率问题problem of the change rate of a function 导函数derived function左导数left-hand derivative右导数right-hand derivative单侧导数one-sided derivatives在闭区间[a, b]上可导is derivable on the closed interval [a,b] 切线方程tangent equation角速度angular velocity成本函数cost function边际成本marginal cost链式法则chain rule隐函数implicit function显函数explicit function二阶函数second derivative三阶导数third derivative高阶导数nth derivative莱布尼茨公式Leibniz formula对数求导法log- derivative参数方程parametric equation相关变化率correlative change rata微分differential可微的differentiable函数的微分differential of function自变量的微分differential of independent variable微商differential quotient间接测量误差indirect measurement error绝对误差absolute error相对误差relative error第三章微分中值定理与导数的应用Chapter3 Mean Value Theorem ofDifferentials and the Application ofDerivatives罗马定理Rolle’s theorem费马引理Fermat’s lemma拉格朗日中值定理Lagrange’s mean value theorem驻点stationary point稳定点stable point 临界点critical point辅助函数auxiliary function拉格朗日中值公式Lagrange’s mean value formula柯西中值定理Cauchy’s mean value theorem 洛必达法则L’Hospital’s Rule0/0型不定式indeterminate form of type 0/0 不定式indeterminate form泰勒中值定理Taylor’s mean value theorem 泰勒公式T aylorformula余项remainder term拉格朗日余项Lagrange remainder term麦克劳林公式Ma claurin’s formula佩亚诺公式Peano remainder term凹凸性concavity凹向上的concave upward, cancave up凹向下的，向上凸的concave downward’ concave down 拐点inflection point函数的极值extremum of function极大值local(relative) maximum最大值global(absolute) mximum极小值local(relative) minimum最小值global(absolute) minimum目标函数objective function曲率curvature弧微分arc differential平均曲率average curvature曲率园circle of curvature曲率中心center of curvature曲率半径radius of curvature渐屈线evolute渐伸线involute根的隔离isolation of root隔离区间isolation interval切线法tangent line method第四章不定积分Chapter4 Indefinite Integrals原函数primitive function(anti-derivative)积分号sign of integration被积函数integrand积分变量integral variable积分曲线integral curve积分表table of integrals换元积分法integration by substitution分部积分法integration by parts分部积分公式formula of integration by parts 有理函数rational function真分式proper fraction假分式improper fraction第五章定积分Chapter5 Definite Integrals曲边梯形trapezoid with曲边curve edge窄矩形narrow rectangle曲边梯形的面积area of trapezoid with curved edge积分下限lower limit of integral积分上限upper limit of integral积分区间integral interval分割partition积分和integral sum可积integrable矩形法rectangle method积分中值定理mean value theorem of integrals函数在区间上的平均值average value of a function on an integvals牛顿－莱布尼茨公式Newton-Leibniz formula微积分基本公式fundamental formula of calculus换元公式formula for integration by substitution递推公式recurrence formula反常积分improper integral 反常积分发散the improper integral is divergent反常积分收敛the improper integral is convergent无穷限的反常积分improper integral on an infinite interval无界函数的反常积分improper integral of unbounded functions绝对收敛absolutely convergent第六章定积分的应用Chapter6 Applications of the DefiniteIntegrals元素法the element method面积元素element of area平面图形的面积area of a luane figure直角坐标又称“笛卡儿坐标(Cartesian coordinates)”极坐标polar coordinates抛物线parabola椭圆ellipse旋转体的面积volume of a solid of rotation旋转椭球体ellipsoid of revolution, ellipsoid of rotation曲线的弧长arc length of acurve可求长的rectifiable光滑smooth功work水压力water pressure引力gravitation变力variable force第七章空间解析几何与向量代数Chapter7 Space Analytic Geometry andVector Algebra向量vector自由向量free vector单位向量unit vector零向量zero vector相等equal平行parallel向量的线性运算linear poeration of vector三角法则triangle rule平行四边形法则parallelogram rule交换律commutative law结合律associative law负向量negative vector差difference分配律distributive law空间直角坐标系space rectangular coordinates坐标面coordinate plane卦限octant向量的模modulus of vector向量a与b的夹角angle between vector a and b方向余弦direction cosine方向角direction angle向量在轴上的投影projection of a vector onto an axis数量积，外积，叉积scalar product,dot product,inner product 曲面方程equation for a surface球面sphere旋转曲面surface of revolution母线generating line轴axis圆锥面cone顶点vertex旋转单叶双曲面revolution hyperboloids of one sheet旋转双叶双曲面revolution hyperboloids of two sheets柱面cylindrical surface ,cylinder圆柱面cylindrical surface准线directrix抛物柱面parabolic cylinder二次曲面quadric surface 椭圆锥面dlliptic cone椭球面ellipsoid单叶双曲面hyperboloid of one sheet双叶双曲面hyperboloid of two sheets旋转椭球面ellipsoid of revolution椭圆抛物面elliptic paraboloid旋转抛物面paraboloid of revolution双曲抛物面hyperbolic paraboloid马鞍面saddle surface椭圆柱面elliptic cylinder双曲柱面hyperbolic cylinder抛物柱面parabolic cylinder空间曲线space curve空间曲线的一般方程general form equations of a space curve 空间曲线的参数方程parametric equations of a space curve 螺转线spiral螺矩pitch投影柱面projecting cylinder投影projection平面的点法式方程pointnorm form eqyation of a plane法向量normal vector平面的一般方程general form equation of a plane两平面的夹角angle between two planes点到平面的距离distance from a point to a plane空间直线的一般方程general equation of a line in space方向向量direction vector直线的点向式方程pointdirection form equations of a line方向数direction number直线的参数方程parametric equations of a line两直线的夹角angle between two lines垂直perpendicular直线与平面的夹角angle between a line and a planes平面束pencil of planes平面束的方程equation of a pencil of planes 行列式determinant系数行列式coefficient determinant第八章多元函数微分法及其应用Chapter8 Differentiation of Functions ofSeveral Variables and Its Application一元函数function of one variable多元函数function of several variables内点interior point外点exterior point边界点frontier point,boundary point聚点point of accumulation开集openset闭集closed set连通集connected set开区域open region闭区域closed region有界集bounded set无界集unbounded setn维空间n-dimentional space二重极限double limit多元函数的连续性continuity of function of seveal连续函数continuous function不连续点discontinuity point一致连续uniformly continuous偏导数partial derivative对自变量x的偏导数partial derivative with respect to independent variable x高阶偏导数partial derivative of higher order 二阶偏导数second order partial derivative混合偏导数hybrid partial derivative全微分total differential偏增量oartial increment偏微分partial differential 全增量total increment可微分differentiable必要条件necessary condition充分条件sufficient condition叠加原理superpostition principle全导数total derivative中间变量intermediate variable隐函数存在定理theorem of the existence of implicit function 曲线的切向量tangent vector of a curve法平面normal plane向量方程vector equation向量值函数vector-valued function切平面tangent plane法线normal line方向导数directional derivative梯度gradient数量场scalar field梯度场gradient field向量场vector field势场potential field引力场gravitational field引力势gravitational potential曲面在一点的切平面tangent plane to a surface at a point曲线在一点的法线normal line to a surface at a point无条件极值unconditional extreme values条件极值conditional extreme values拉格朗日乘数法Lagrange multiplier method 拉格朗日乘子Lagrange multiplier经验公式empirical formula最小二乘法method of least squares均方误差mean square error第九章重积分Chapter9 Multiple Integrals二重积分double integral可加性additivity累次积分iterated integral体积元素volume element三重积分triple integral直角坐标系中的体积元素volume element in rectangular coordinate system柱面坐标cylindrical coordinates柱面坐标系中的体积元素volume element in cylindrical coordinate system球面坐标spherical coordinates球面坐标系中的体积元素volume element in spherical coordinate system反常二重积分improper double integral曲面的面积area of a surface质心centre of mass静矩static moment密度density形心centroid转动惯量moment of inertia参变量parametric variable第十章曲线积分与曲面积分Chapter10 Line (Curve) Integrals andSurface Integrals对弧长的曲线积分line integrals with respect to arc hength第一类曲线积分line integrals of the first type对坐标的曲线积分line integrals with respect to x,y,and z第二类曲线积分line integrals of the second type有向曲线弧directed arc单连通区域simple connected region复连通区域complex connected region格林公式Green formula第一类曲面积分surface integrals of the first type对面的曲面积分surface integrals with respect to area 有向曲面directed surface对坐标的曲面积分surface integrals with respect to coordinate elements第二类曲面积分surface integrals of the second type有向曲面元element of directed surface高斯公式gauss formula拉普拉斯算子Laplace operator格林第一公式Green’s first formula通量flux散度divergence斯托克斯公式Stokes formula环流量circulation旋度rotation,curl第十一章无穷级数Chapter11 Infinite Series一般项general term部分和partial sum余项remainder term等比级数geometric series几何级数geometric series公比common ratio调和级数harmonic series柯西收敛准则Cauchy convergence criteria, Cauchy criteria for convergence正项级数series of positive terms达朗贝尔判别法D’Alembert test柯西判别法Cauchy test交错级数alternating series绝对收敛absolutely convergent条件收敛conditionally convergent柯西乘积Cauchy product函数项级数series of functions发散点point of divergence收敛点point of convergence收敛域convergence domain和函数sum function幂级数power series幂级数的系数coeffcients of power series阿贝尔定理Abel Theorem收敛半径radius of convergence收敛区间interval of convergence泰勒级数Taylor series麦克劳林级数Maclaurin series二项展开式binomial expansion近似计算approximate calculation舍入误差round-off error,rounding error欧拉公式Euler’s formula魏尔斯特拉丝判别法Weierstrass test三角级数trigonometric series振幅amplitude角频率angular frequency初相initial phase矩形波square wave谐波分析harmonic analysis直流分量direct component基波fundamental wave二次谐波second harmonic三角函数系trigonometric function system傅立叶系数Fourier coefficient傅立叶级数Forrier series周期延拓periodic prolongation正弦级数sine series余弦级数cosine series奇延拓odd prolongation偶延拓even prolongation傅立叶级数的复数形式complex form of Fourier series第十二章微分方程Chapter12 Differential Equation解微分方程solve a differential equation常微分方程ordinary differential equation偏微分方程partial differential equation,PDE 微分方程的阶order of a differential equation 微分方程的解solution of adifferential equation 微分方程的通解general solution of a differential equation初始条件initial condition微分方程的特解particular solution of a differential equation 初值问题initial value problem微分方程的积分曲线integral curve of a differential equation 可分离变量的微分方程variable separable differential equation 隐式解implicit solution隐式通解inplicit general solution衰变系数decay coefficient衰变decay齐次方程homogeneous equation一阶线性方程linear differential equation of first order非齐次non-homogeneous齐次线性方程homogeneous linear equation 非齐次线性方程non-homogeneous linear equation常数变易法method of variation of constant 暂态电流transient state current稳态电流steady state current伯努利方程Bernoulli equation全微分方程total differential equation积分因子integrating factor高阶微分方程differential equation of higher order悬链线catenary高阶线性微分方程linear differential equation of higher order 自由振动的微分方程differential equation of free vibration强迫振动的微分方程differential equation of forced oscillation 串联电路的振荡方程oscillation equation of series circuit二阶线性微分方程second order lineardifferential equation线性相关linearly dependence线性无关linearly independence二阶常系数齐次线性微分方程second order homogeneous linear differential equation with constant coefficient二阶变系数齐次线性微分方程second order homogeneous linear differential equation with variable coefficient特征方程characteristic equation无阻尼自由振动的微分方程differential equation of free vibration with zero damping 固有频率natural frequency 简谐振动simple harmonic oscillation,simple harmonic vibration 微分算子differential operator待定系数法method of undetermined coefficient共振现象resonance phenomenon欧拉方程Euler equation幂级数解法power series solution数值解法numerial solution勒让德方程Legendre equation微分方程组system of differential equations常系数线性微分方程组system of linear differential equations with constant coefficientV、X、Z:Value of function ：函数值Variable ：变数Vector ：向量Velocity ：速度Vertical asymptote ：垂直渐近线Volume ：体积X-axis ：x轴x-coordinate ：x坐标x-intercept ：x截距Zero vector ：函数的零点Zeros of a polynomial ：多项式的零点T:Tangent function ：正切函数Tangent line ：切线Tangent plane ：切平面Tangent vector ：切向量Total differential ：全微分Trigonometric function ：三角函数Trigonometric integrals ：三角积分Trigonometric substitutions ：三角代换法Tripe integrals ：三重积分S:Saddle point ：鞍点Scalar ：纯量Secant line ：割线Second derivative ：二阶导数Second Derivative Test ：二阶导数试验法Second partial derivative ：二阶偏导数Sector ：扇形Sequence ：数列Series ：级数Set ：集合Shell method ：剥壳法Sine function ：正弦函数Singularity ：奇点Slant asymptote ：斜渐近线Slope ：斜率Slope-intercept equation of a line ：直线的斜截式Smooth curve ：平滑曲线Smooth surface ：平滑曲面Solid of revolution ：旋转体Space ：空间Speed ：速率Spherical coordinates ：球面坐标Squeeze Theorem ：夹挤定理Step function ：阶梯函数Strictly decreasing ：严格递减Strictly increasing ：严格递增Sum ：和Surface ：曲面Surface integral ：面积分Surface of revolution ：旋转曲面Symmetry ：对称R:Radius of convergence ：收敛半径Range of a function ：函数的值域Rate of change ：变化率Rational function ：有理函数Rationalizing substitution ：有理代换法Rational number ：有理数Real number ：实数Rectangular coordinates ：直角坐标Rectangular coordinate system ：直角坐标系Relative maximum and minimum ：相对极大值与极小值Revenue function ：收入函数Revolution , solid of ：旋转体Revolution , surface of ：旋转曲面Riemann Sum ：黎曼和Riemannian geometry ：黎曼几何Right-hand derivative ：右导数Right-hand limit ：右极限Root ：根P、Q:Parabola ：拋物线Parabolic cylinder ：抛物柱面Paraboloid ：抛物面Parallelepiped ：平行六面体Parallel lines ：并行线Parameter ：参数Partial derivative ：偏导数Partial differential equation ：偏微分方程Partial fractions ：部分分式Partial integration ：部分积分Partiton ：分割Period ：周期Periodic function ：周期函数Perpendicular lines ：垂直线Piecewise defined function 分段定义函数Plane ：平面Point of inflection ：反曲点Polar axis ：极轴Polar coordinate ：极坐标Polar equation ：极方程式Pole ：极点Polynomial ：多项式Positive angle ：正角Point-slope form ：点斜式Power function ：幂函数Product ：积Quadrant ：象限Quotient Law of limit 极限的商定律Quotient Rule ：商定律M、N、O:Maximum and minimum values ：极大与极小值Mean Value Theorem ：均值定理Multiple integrals ：重积分Multiplier ：乘子Natural exponential function 自然指数函数Natural logarithm function 自然对数函数Natural number ：自然数Normal line ：法线Normal vector ：法向量Number ：数Octant ：卦限Odd function ：奇函数One-sided limit ：单边极限Open interval ：开区间Optimization problems ：最佳化问题Order ：阶Ordinary differential equation ：常微分方程Origin ：原点Orthogonal ：正交的L:Laplace transform ：Leplace 变换Law of Cosines ：余弦定理Least upper bound ：最小上界Left-hand derivative ：左导数Left-hand limit ：左极限Lemniscate ：双钮线Length ：长度Level curve ：等高线L'Hospital's rule 洛必达法则Limacon ：蚶线Limit ：极限Linear approximation：线性近似Linear equation ：线性方程式Linear function ：线性函数Linearity ：线性Linearization ：线性化Line in the plane ：平面上之直线Line in space ：空间之直线Lobachevski geometry 罗巴切夫斯基几何Local extremum ：局部极值Local maximum and minimum 局部极大值与极小值Logarithm ：对数Logarithmic function ：对数函数I:Implicit differentiation ：隐求导法Implicit function ：隐函数Improper integral ：瑕积分Increasing/Decreasing Test 递增或递减试验法Increment ：增量Increasing Function ：增函数Indefinite integral ：不定积分Independent variable ：自变数Indeterminate from ：不定型Inequality ：不等式Infinite point ：无穷极限Infinite series ：无穷级数Inflection point ：反曲点Instantaneous velocity ：瞬时速度Integer ：整数Integral ：积分Integrand ：被积分式Integration ：积分Integration by part ：分部积分法Intercepts ：截距Intermediate value of Theorem 中间值定理Interval ：区间Inverse function ：反函数Inverse trigonometric function反三角函数Iterated integral ：逐次积分H:Higher mathematics 高等数学/高数E、F、G、H:Ellipse ：椭圆Ellipsoid ：椭圆体Epicycloid ：外摆线Equation ：方程式Even function ：偶函数Expected Valued ：期望值Exponential Function ：指数函数Exponents , laws of ：指数率Extreme value ：极值Extreme Value Theorem ：极值定理Factorial ：阶乘First Derivative Test ：一阶导数试验法First octant ：第一卦限Focus ：焦点Fractions ：分式Function ：函数Fundamental Theorem of Calculus ：微积分基本定理Geometric series ：几何级数Gradient ：梯度Graph ：图形Green Formula ：格林公式Half-angle formulas ：半角公式Harmonic series ：调和级数Helix ：螺旋线Higher Derivative ：高阶导数Horizontal asymptote ：水平渐近线Horizontal line ：水平线Hyperbola ：双曲线Hyper boloid ：双曲面D:Decreasing function ：递减函数Decreasing sequence ：递减数列Definite integral ：定积分Degree of a polynomial ：多项式之次数Density ：密度Derivative ：导数of a composite function ：复合函数之导数of a constant function ：常数函数之导数directional ：方向导数domain of ：导数之定义域of exponential function ：指数函数之导数higher ：高阶导数partial ：偏导数of a power function ：幂函数之导数of a power series ：羃级数之导数of a product ：积之导数of a quotient ：商之导数as a rate of change ：导数当作变率right-hand ：右导数second ：二阶导数as the slope of a tangent 导数看成切线之斜率Determinant ：行列式Differentiable function 可导函数Differential ：微分Differential equation ：微分方程partial ：偏微分方程Differentiation ：求导法implicit ：隐求导法partial ：偏微分法term by term ：逐项求导法Directional derivatives ：方向导数Discontinuity ：不连续性Disk method ：圆盘法Distance ：距离Divergence ：发散Domain ：定义域Dot product ：点积Double integral ：二重积分change of variable in二重积分之变数变换in polar coordinates ：极坐标二重积分C:Calculus ：微积分differential ：微分学integral ：积分学Cartesian coordinates ：笛卡儿坐标一般指直角坐标Cartesian coordinates system 笛卡儿坐标系Cauch’s Mean Value Theorem 柯西均值定理Chain Rule ：连锁律Change of variables ：变数变换Circle ：圆Circular cylinder ：圆柱Closed interval ：封闭区间Coefficient ：系数Composition of function ：函数之合成Compound interest ：复利Concavity ：凹性Conchoid ：蚌线Cone ：圆锥Constant function ：常数函数Constant of integration ：积分常数Continuity ：连续性at a point ：在一点处之连续性of a function ：函数之连续性on an interval ：在区间之连续性from the left ：左连续from the right ：右连续Continuous function ：连续函数Convergence ：收敛interval of ：收敛区间radius of ：收敛半径Convergent sequence ：收敛数列series ：收敛级数Coordinate：s：坐标Cartesian ：笛卡儿坐标cylindrical ：柱面坐标polar ：极坐标rectangular ：直角坐标spherical ：球面坐标Coordinate axes ：坐标轴Coordinate planes ：坐标平面Cosine function ：余弦函数Critical point ：临界点Cubic function ：三次函数Curve ：曲线Cylinder：圆柱Cylindrical Coordinates ：圆柱坐标A、B:Absolute convergence ：绝对收敛Absolute extreme values ：绝对极值Absolute maximum and minimum 绝对极大与极小Absolute value ：绝对值Absolute value function ：绝对值函数Acceleration ：加速度Antiderivative ：反导数Approximate integration ：近似积分Approximation ：逼近法by differentials ：用微分逼近linear ：线性逼近法by Simpson’s Rule ：Simpson法则逼近法by the Trapezoidal Rule 梯形法则逼近法Arbitrary constant ：任意常数Arc length ：弧长Area ：面积under a curve ：曲线下方之面积between curves ：曲线间之面积in polar coordinates ：极坐标表示之面积of a sector of a circle ：扇形之面积of a surface of a revolution 旋转曲面之面积Asymptote ：渐近线horizontal ：水平渐近线slant ：斜渐近线vertical ：垂直渐近线Average speed ：平均速率Average velocity ：平均速度Axes, coordinate ：坐标轴Axes of ellipse ：椭圆之轴Binomial series ：二项级数微积分专有名词中英文对照absolutely convergent 绝对收敛absolute value 绝对值algebraic function 代数函数analytic geometry 解析几何antiderivative 不定积分approximate integration 近似积分approximation 近似法、逼近法arbitrary constant 任意常数arithmetic series/progression (AP)算数级数asymptotes (vertical and horizontal)(垂直/水平)渐近线average rate of change 平均变化率base 基数binomial theorem 二项式定理，二项展开式Cartesian coordinates 笛卡儿坐标(一般指直角坐标)Cartesian coordinates system 笛卡儿坐标系Cauch’s Mean Value Theorem 柯西均值定理chain rule 链式求导法则calculus 微积分学closed interval integral 闭区间积分coefficient 系数composite function 复合函数conchoid 蚌线continuity (函数的)连续性concavity (函数的)凹凸性conditionally convergent 有条件收敛continuity 连续性critical point 临界点cubic function 三次函数cylindrical coordinates 圆柱坐标decreasing function 递减函数decreasing sequence 递减数列definite integral 定积分derivative 导数determinant 行列式differential coefficient 微分系数differential equation 微分方程directional derivative 方向导数discontinuity 不连续性discriminant (二次函数)判别式disk method 圆盘法divergence 散度divergent 发散的domain 定义域dot product 点积double integral 二重积分ellipse 椭圆ellipsoid 椭圆体epicycloid 外摆线Euler's method (BC)欧拉法expected valued 期望值exponential function 指数函数extreme value heorem 极值定理factorial 阶乘finite series 有限级数fundamental theorem of calculus 微积分基本定理geometric series/progression (GP)几何级数gradient 梯度Green formula 格林公式half-angle formulas 半角公式harmonic series 调和级数helix 螺旋线higher derivative 高阶导数horizontal asymptote 水平渐近线horizontal line 水平线hyperbola 双曲线hyper boloid 双曲面implicit differentiation 隐函数求导implicit function 隐函数improper integral 广义积分、瑕积分increment 增量increasing function 增函数indefinite integral 不定积分independent variable 自变数inequality 不等式ndeterminate form 不定型infinite point 无穷极限infinite series 无穷级数infinite series 无限级数inflection point (POI) 拐点initial condition 初始条件instantaneous rate of change 瞬时变化率integrable 可积的integral 积分integrand 被积分式integration 积分integration by part 分部积分法intercept 截距intermediate value of Theorem ：中间值定理inverse function 反函数irrational function 无理函数iterated integral 逐次积分Laplace transform 拉普拉斯变换law of cosines 余弦定理least upper bound 最小上界left-hand derivative 左导数left-hand limit 左极限L'Hospital's rule 洛必达法则limacon 蚶线linear approximation 线性近似法linear equation 线性方程式linear function 线性函数linearity 线性linearization 线性化local maximum 极大值local minimum 极小值logarithmic function 对数函数MacLaurin series 麦克劳林级数maximum 最大值mean value theorem (MVT)中值定理minimum 最小值method of lagrange multipliers 拉格朗日乘数法modulus 绝对值multiple integral 多重积分multiple 倍数multiplier 乘子octant 卦限open interval integral 开区间积分optimization 优化法，极值法origin 原点orthogonal 正交parametric equation (BC)参数方程partial derivative 偏导数partial differential equation 偏微分方程partial fractions 部分分式piece-wise function 分段函数parabola 抛物线parabolic cylinder 抛物柱面paraboloid ：抛物面parallelepiped 平行六面体parallel lines 并行线parameter ：参数partial integration 部分积分partiton ：分割period ：周期periodic function 周期函数perpendicular lines 垂直线piecewise defined function 分段定义函数plane 平面point of inflection 反曲点point-slope form 点斜式polar axis 极轴polar coordinates 极坐标polar equation 极坐标方程pole 极点polynomial 多项式power series 幂级数product rule 积的求导法则quadrant 象限quadratic functions 二次函数quotient rule 商的求导法则radical 根式radius of convergence 收敛半径range 值域(related) rate of change with time (时间)变化率rational function 有理函数reciprocal 倒数remainder theorem 余数定理Riemann sum 黎曼和Riemannian geometry 黎曼几何right-hand limit 右极限Rolle's theorem 罗尔(中值)定理root 根rotation 旋转secant line 割线second derivative 二阶导数second derivative test 二阶导数试验法second partial derivative 二阶偏导数series 级数shell method (积分)圆筒法sine function 正弦函数singularity 奇点slant 母线slant asymptote 斜渐近线slope 斜率slope-intercept equation of a line 直线的斜截式smooth curve 平滑曲线smooth surface 平滑曲面solid of revolution 旋转体symmetry 对称性substitution 代入法、变量代换tangent function 正切函数tangent line 切线tangent plane 切(平)面tangent vector 切矢量taylor's series 泰勒级数three-dimensional analytic geometry 空间解析几何total differentiation 全微分trapezoid rule 梯形(积分)法则。

数学专业英语课文翻译

1-A：什么是数学数学来源于人类的社会实践，包括工农业的劳动，商业、军事和科学技术研究等活动。

反过来，数学服务于实践并在所有领域扮演一个重要的角色。

没有数学的应用，现代化科学和技术的分支都不能有规律的发展。

从早期人类的需求引出了数和形状。

然后，几何学因测量陆续的发展出来，三角学来自于勘探问题。

为了处理一些更复杂的实践问题，人们建立了方程，通过求解方程的未知数，从而代数学出现了。

17世纪之前，人们局限于初等数学，例如几何、三角和代数，那些只考虑常数。

17世纪工业的迅速发展促进了经济学和科技的发展，并且我们需要处理变量。

从常数到变量的跳跃带来了两个属于高等数学的新的数学分支，解析几何和微积分学。

现在，高等数学中有了许多分支，数学分析、高等代数、微分方程、函数论等。

数学家们研究概念和命题。

公理、公社、定义和定理都是命题。

符号是一种特别并且很重要的数学工具，它常用于表示概念和命题。

公式、图形和表格充满着不同的符号。

阿拉伯数字1,2,3,4,5,6,7,8,9,0和加”+”减”-”乘”*”除”/”等号”=”使我们最熟悉的数学符号。

主要通过逻辑推导和计算来获得数学结论。

在数学史的很长的时期内，逻辑推论一直占据数学方法的中心地位。

现在，自从电子计算机迅速发展和广泛应用，计算的角色越来越重要。

现在，计算不仅用来处理信息与数据，而且用来完成一些在以前只能靠逻辑推理来做的工作，例如证明大多数的几何定理。

1-B：等式等式是关于两个数或数的符号相等的一种陈述。

因此a(a-5)=a^2-5a和x-3=5是等式。

等式有两种，恒等式和条件等式。

算术和代数恒等式是等式。

这种等式的两端要么一样，要么经过执行指定的运算后变成一样。

因此12-2=2+8，(m-n)(m+n)=m^2-n^2是恒等式。

含有字母的恒等式对其中字母的任何一组数值都成立。

因此恒等式x(a+2)=ax+2x变成3(7+2)=21+6或27=27，比如当x=3和a=7。

数学专业英语(Doc版).10

学专业英语－How to Write Mathematics?How to Write Mathematics?------ Honesty is the Best PolicyThe purpose of using good mathematical language is, of course, to make the u nderstanding of the subject easy for the reader, and perhaps even pleasant. The style should be good not in the sense of flashy brilliance, but good in the se nse of perfect unobtrusiveness. The purpose is to smooth the reader’s wanted, not pedantry; understanding, not fuss.The emphasis in the preceding paragraph, while perhaps necessary, might see m to point in an undesirable direction, and I hasten to correct a possible misin terpretation. While avoiding pedantry and fuss, I do not want to avoid rigor an d precision; I believe that these aims are reconcilable. I do not mean to advise a young author to be very so slightly but very very cleverly dishonest and to gloss over difficulties. Sometimes, for instance, there may be no better way t o get a result than a cumbersome computation. In that case it is the author’s duty to carry it out, in public; the he can do to alleviate it is to extend his s ympathy to the reader by some phrase such as “unfortunately the only known proof is the following cumbersome computation.”Here is the sort of the thing I mean by less than complete honesty. At a certa in point, having proudly proved a proposition P, you feel moved to say: “Not e, however, that p does not imply q”, and then, thinking that you’ve done a good expository job, go happily on to other things. Your motives may be per fectly pure, but the reader may feel cheated just the same. If he knew all abo ut the subject, he wouldn’t be reading you; for him the nonimplication is, qui te likely, unsupported. Is it obvious? (Say so.) Will a counterexample be suppl ied later? (Promise it now.) Is it a standard present purposes irrelevant part of the literature? (Give a reference.) Or, horrible dictum, do you merely mean th at you have tried to derive q from p, you failed, and you don’t in fact know whether p implies q? (Confess immediately.) any event: take the reader into y our confidence.There is nothing wrong with often derided “obvious”and “easy to see”, b ut there are certain minimal rules to their use. Surely when you wrote that so mething was obvious, you thought it was. When, a month, or two months, or six months later, you picked up the manuscript and re-read it, did you still thi nk that something was obvious? (A few months’ripening always improves ma nuscripts.) When you explained it to a friend, or to a seminar, was the someth ing at issue accepted as obvious? (Or did someone question it and subside, mu ttering, when you reassured him? Did your assurance demonstration or intimidation?) the obvious answers to these rhetorical questions are among the rules th at should control the use of “obvious”. There is the most frequent source o f mathematical error: make that the “obvious”is true.It should go without saying that you are not setting out to hide facts from the reader: you are writing to uncover them. What I am saying now is that you should not hide the status of your statements and your attitude toward them eit her. Whenever you tell him something, tell him where it stands: this has been proved, that hasn’t, this will be proved, that won’t. Emphasize the importan t and minimize the trivial. The reason saying that they are obvious is to put t hem in proper perspecti e for the uninitiated. Even if your saying so makes an occasional reader angry at you, a good purpose is served by your telling him how you view the matter. But, of course, you must obey the rules. Don’t le t the reader down; he wants to believe in you. Pretentiousness, bluff, and conc ealment may not get caught out immediately, but most readers will soon sense that there is something wrong, and they will blame neither the facts nor them selves, but quite properly, the author. Complete honesty makes for greatest clar ity.---------Paul R.Haqlmosvocabularyflashy 一闪的 counter-example 反例unobtrusiveness 谦虚dictum 断言；格言forestall 阻止，先下手deride嘲弄anticipate 预见 subside沉静pedantry 迂腐；卖弄学问 mutter出怨言，喃喃自语fuss 小题大做 intimidation威下reconcilable 使一致的 rhetorical合符修辞学的gloss 掩饰 pretentiousness自命不凡alleviate 减轻，缓和bluff 欺骗implication 包含，含意concealment隐匿notes1. 本课文选自美国数学学会出版的小册子How to write mathematics 中Paul R.Halmos. 的文章第9节2. The purpose is smooth the reader’way, to anticipates his difficulties and to forestall them. Clarit y is what’s wanted, not pedantry; understanding, not fuss.意思是：目的是为读者扫清阅读上的障碍，即预先设想读者会遇到什么困难，并力求避免出现这类困难。