43 Rational Functions I43我有理函数
AP微积分单词

AP CACULUS WORDS absolutely convergent ,adj绝对收敛antiderivative ,n不定积分binomial theorem ,n二项式定理,二项展开式chain rule ,n链规则calculus ,n微积分学conditionally convergent ,adj条件收敛continuity ,n连续converge ,v收敛definite integral ,n定积分derivative ,n导数differential coefficient ,n微分differential equation ,n微分方程directional derivative ,n方向导数diverge ,v发散divergence ,n散度gradient ,n梯度implicit differentiation ,n隐函数微分implicit function ,n隐函数improper integral ,n广义积分integrable ,adj可积的integral ,n积分integration by parts ,n部分积分法left-hand limit ,n左极限limit ,n极限local maximum ,n极大值local minimum ,n极小值method of lagrange multipliers ,n拉格朗日乘数法multiple integral ,n多重积分partial derivative ,n偏导数polar coordinates ,n极坐标power series ,n幂级数right-hand limit ,n右极限rotation ,n旋度series ,n级数substitution rule ,n替代法则table of integrals ,n积分表taylor’s formula ,n泰勒公式three-dimensional analytic geometry ,n空间解析几何total differential ,n全微分trigonometric substitution ,n三角替代法absolutely convergent绝对收敛absolute value绝对值algebraic function代数函数analytic geometry解析几何antiderivative不定积分approximate integration近似积分approximation近似法、逼近法arbitrary constant任意常数arithmetic series/progression (AP)算数级数asymptotes (vertical and horizontal)(垂直/水平)渐近线average rate of change平均变化率base基数binomial theorem二项式定理,二项展开式Cartesian coordinates笛卡儿坐标(一般指直角坐标) Cartesian coordinates system笛卡儿坐标系Cauch’s Mean Value Theorem柯西均值定理chain rule链式求导法则calculus微积分学closed interval integral闭区间积分coefficient系数conchoid蚌线continuity (函数的)连续性concavity (函数的)凹凸性conditionally convergent有条件收敛continuity连续性critical point临界点cubic function三次函数cylindrical coordinates圆柱坐标decreasing function递减函数decreasing sequence递减数列definite integral定积分derivative导数determinant行列式differential coefficient微分系数differential equation微分方程directional derivative方向导数discontinuity不连续性discriminant (二次函数)判别式disk method圆盘法divergence散度divergent发散的domain定义域dot product点积double integral二重积分ellipse椭圆ellipsoid椭圆体epicycloid外摆线Euler's method (BC)欧拉法expected valued期望值exponential function指数函数extreme value heorem极值定理factorial阶乘finite series有限级数fundamental theorem of calculus微积分基本定理geometric series/progression (GP)几何级数gradient梯度Green formula格林公式half-angle formulas半角公式harmonic series调和级数helix螺旋线higher derivative高阶导数horizontal asymptote水平渐近线horizontal line水平线hyperbola双曲线hyper boloid双曲面implicit differentiation隐函数求导implicit function隐函数improper integral广义积分、瑕积分increment增量increasing function增函数indefinite integral不定积分independent variable自变数inequality不等式ndeterminate form不定型infinite point无穷极限infinite series无穷级数infinite series无限级数inflection point (POI)拐点initial condition初始条件instaneous rate of change瞬时变化率integrable可积的integral积分integrand被积分式integration积分integration by part分部积分法intercept截距intermediate value of Theorem:中间值定理inverse function反函数irrational function无理函数iterated integral逐次积分Laplace transform拉普拉斯变换law of cosines余弦定理least upper bound最小上界left-hand derivative左导数left-hand limit左极限L'Hospital's rule洛必达法则limacon蚶线linear approximation线性近似法linear equation线性方程式linear function线性函数linearity线性linearization线性化local maximum极大值local minimum极小值logarithmic function对数函数MacLaurin series麦克劳林级数maximum最大值mean value theorem (MVT)中值定理minimum最小值method of lagrange multipliers拉格朗日乘数法modulus绝对值multiple integral多重积分multiple倍数multiplier乘子octant卦限open interval integral开区间积分optimization优化法,极值法origin原点orthogonal正交parametric equation (BC)参数方程partial derivative偏导数partial differential equation偏微分方程partial fractions部分分式piece-wise function分段函数parabola抛物线parabolic cylinder抛物柱面paraboloid:抛物面parallepiped平行六面体parallel lines并行线parameter:参数partial integration部分积分partiton:分割period:周期periodic function周期函数perpendicular lines垂直线piecewise defined function分段定义函数plane平面point of inflection反曲点point-slope form点斜式polar axis极轴polar coordinates极坐标polar equation极坐标方程pole极点polynomial多项式power series幂级数product rule积的求导法则quadrant象限quadratic functions二次函数quotient rule商的求导法则radical根式radius of convergence收敛半径range值域(related) rate of change with time (时间)变化率rational function有理函数reciprocal倒数remainder theorem余数定理Riemann sum黎曼和Riemannian geometry黎曼几何right-hand limit右极限Rolle's theorem罗尔(中值)定理root根rotation旋转secant line割线second derivative二阶导数second derivative test二阶导数试验法second partial derivative二阶偏导数series级数shell method (积分)圆筒法sine function正弦函数singularity奇点slant母线slant asymptote斜渐近线slope斜率slope-intercept equation of a line直线的斜截式smooth curve平滑曲线smooth surface平滑曲面solid of revolution旋转体symmetry对称性substitution代入法、变量代换tangent function正切函数tangent line切线tangent plane切(平)面tangent vector切矢量taylor's series泰勒级数three-dimensional analytic geometry空间解析几何total differentiation全微分trapezoid rule梯形(积分)法则。
数学总结 Function 函数
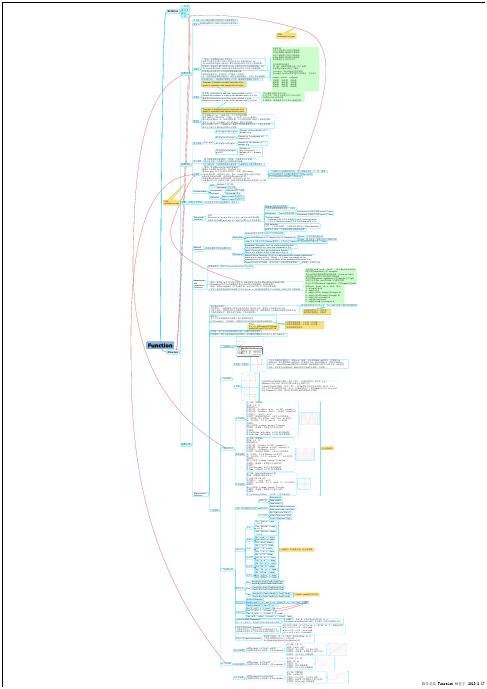
余割函数 Cosecant csc
7
表达式
语言描述
a/h ∠A的对边比斜边
b/h ∠A的邻边比斜边
a/b ∠A的对边比邻边
b/a ∠A的邻边比对边
h/b ∠A的斜边比邻边
h/a ∠A的斜边比对边
正弦线、余弦线
引入有向线段的概念后,如果x>0,如图,有向线段OM与x轴同向,其数量为x, 如果x<0,有向线段OM与x轴反向,其数量也为x,故总有OM=x。同理可知MP=y 所以有,sinα=MP,cosα=OM即有向线段MP、OM的数量分别等于α的正弦、α的余弦。 因此,我们把有向线段MP,OM分别叫作角α的正弦线、余弦线。
若m≥n,做长除式,商即为水平/斜渐近线
Hole:当分子被分母中的factor整除时,分母中这个factor对应的x的值的位置会有一个hole
Remainder Theorems: Let f be a polynomial function. If f(x) is divided by x-c, then the remainder is f(c)
如果a>0,且a≠1,M>0,N>0,那么: 1、a^log(a) N=N 2、log(a) a=1 3、log(a) (M·N)=log(a) M+log(a) N 4、log(a) (M÷N)=log(a) M-log(a) N 5、log(a) M^n=nlog(a) M 6、log(a)b*log(b)a=1 7、log(a) b=log (c) b/log (c) a
奇偶性
奇函数:A function is odd if for every number x in its
domain the number -x is also in the domain and f(-x)=-f(x)
英汉数学物理常用词汇

Cauchy's integral formula,科西积分公式
Liouville's theorem,刘维尔定理
Sequence,序列
sequence of number,数列
sequence of function,函数列
derivative,导数
partial derivative,偏导数
higher derivative,高阶导数
derivative of order n/nth order derivative,n阶导数
analytic function,解析函数
regular function,正则函数
transcendented function,超越函数
hyperbolic function,双曲函数
anti-hyperbolic function,反双曲函数
trigonometric function,三角函数
anti-trigonometric function,反三角函数
analyticity,解析性
general term,通项
series,级数
series of numbers,数项级数
series of functions/functional series,函项级数
number of term,项数
infinite series,无穷级数
partial sum,部分和
remainder term,余项
multiply valued function,多值函数
simple function,单叶函数
高等数学专业名词中英文对照(全面)

微积分英文词汇,高数名词中英文对照,高等数学术语英语翻译一览关键词:微积分英文,高等数学英文翻译,高数英语词汇来源:上海外教网| 发布日期:2008-05—16 17:12V、X、Z:Value of function :函数值Variable :变数Vector :向量Velocity :速度Vertical asymptote :垂直渐近线Volume :体积X-axis :x轴x-coordinate :x坐标x-intercept :x截距Zero vector :函数的零点Zeros of a polynomial :多项式的零点T:Tangent function :正切函数Tangent line :切线Tangent plane :切平面Tangent vector :切向量Total differential :全微分Trigonometric function :三角函数Trigonometric integrals :三角积分Trigonometric substitutions :三角代换法Tripe integrals :三重积分S:Saddle point :鞍点Scalar :纯量Secant line :割线Second derivative :二阶导数Second Derivative Test :二阶导数试验法Second partial derivative :二阶偏导数Sector :扇形Sequence :数列Series :级数Set :集合Shell method :剥壳法Sine function :正弦函数Singularity :奇点Slant asymptote :斜渐近线Slope :斜率Slope-intercept equation of a line :直线的斜截式Smooth curve :平滑曲线Smooth surface :平滑曲面Solid of revolution :旋转体Space :空间Speed :速率Spherical coordinates :球面坐标Squeeze Theorem :夹挤定理Step function :阶梯函数Strictly decreasing :严格递减Strictly increasing :严格递增Sum :和Surface :曲面Surface integral :面积分Surface of revolution :旋转曲面Symmetry :对称R:Radius of convergence :收敛半径Range of a function :函数的值域Rate of change :变化率Rational function :有理函数Rationalizing substitution :有理代换法Rational number :有理数Real number :实数Rectangular coordinates :直角坐标Rectangular coordinate system :直角坐标系Relative maximum and minimum :相对极大值与极小值Revenue function :收入函数Revolution ,solid of :旋转体Revolution ,surface of :旋转曲面Riemann Sum :黎曼和Riemannian geometry :黎曼几何Right—hand derivative :右导数Right-hand limit :右极限Root :根P、Q:Parabola :拋物线Parabolic cylinder :抛物柱面Paraboloid :抛物面Parallelepiped :平行六面体Parallel lines :并行线Parameter :参数Partial derivative :偏导数Partial differential equation :偏微分方程Partial fractions :部分分式Partial integration :部分积分Partiton :分割Period :周期Periodic function :周期函数Perpendicular lines :垂直线Piecewise defined function :分段定义函数Plane :平面Point of inflection :反曲点Polar axis :极轴Polar coordinate :极坐标Polar equation :极方程式Pole :极点Polynomial :多项式Positive angle :正角Point-slope form :点斜式Power function :幂函数Product :积Quadrant :象限Quotient Law of limit :极限的商定律Quotient Rule :商定律M、N、O:Maximum and minimum values :极大与极小值Mean Value Theorem :均值定理Multiple integrals :重积分Multiplier :乘子Natural exponential function :自然指数函数Natural logarithm function :自然对数函数Natural number :自然数Normal line :法线Normal vector :法向量Number :数Octant :卦限Odd function :奇函数One—sided limit :单边极限Open interval :开区间Optimization problems :最佳化问题Order :阶Ordinary differential equation :常微分方程Origin :原点Orthogonal :正交的L:Laplace transform :Leplace 变换Law of Cosines :余弦定理Least upper bound :最小上界Left—hand derivative :左导数Left—hand limit :左极限Lemniscate :双钮线Length :长度Level curve :等高线L’Hospital’s rule :洛必达法则Limacon :蚶线Limit :极限Linear approximation:线性近似Linear equation :线性方程式Linear function :线性函数Linearity :线性Linearization :线性化Line in the plane :平面上之直线Line in space :空间之直线Lobachevski geometry :罗巴切夫斯基几何Local extremum :局部极值Local maximum and minimum :局部极大值与极小值Logarithm :对数Logarithmic function :对数函数I:Implicit differentiation :隐求导法Implicit function :隐函数Improper integral :瑕积分Increasing/Decreasing Test :递增或递减试验法Increment :增量Increasing Function :增函数Indefinite integral :不定积分Independent variable :自变数Indeterminate from :不定型Inequality :不等式Infinite point :无穷极限Infinite series :无穷级数Inflection point :反曲点Instantaneous velocity :瞬时速度Integer :整数Integral :积分Integrand :被积分式Integration :积分Integration by part :分部积分法Intercepts :截距Intermediate value of Theorem :中间值定理Interval :区间Inverse function :反函数Inverse trigonometric function :反三角函数Iterated integral :逐次积分H:Higher mathematics 高等数学/高数E、F、G、H:Ellipse :椭圆Ellipsoid :椭圆体Epicycloid :外摆线Equation :方程式Even function :偶函数Expected Valued :期望值Exponential Function :指数函数Exponents ,laws of :指数率Extreme value :极值Extreme Value Theorem :极值定理Factorial :阶乘First Derivative Test :一阶导数试验法First octant :第一卦限Focus :焦点Fractions :分式Function :函数Fundamental Theorem of Calculus :微积分基本定理Geometric series :几何级数Gradient :梯度Graph :图形Green Formula :格林公式Half—angle formulas :半角公式Harmonic series :调和级数Helix :螺旋线Higher Derivative :高阶导数Horizontal asymptote :水平渐近线Horizontal line :水平线Hyperbola :双曲线Hyper boloid :双曲面D:Decreasing function :递减函数Decreasing sequence :递减数列Definite integral :定积分Degree of a polynomial :多项式之次数Density :密度Derivative :导数of a composite function :复合函数之导数of a constant function :常数函数之导数directional :方向导数domain of :导数之定义域of exponential function :指数函数之导数higher :高阶导数partial :偏导数of a power function :幂函数之导数of a power series :羃级数之导数of a product :积之导数of a quotient :商之导数as a rate of change :导数当作变率right—hand :右导数second :二阶导数as the slope of a tangent :导数看成切线之斜率Determinant :行列式Differentiable function :可导函数Differential :微分Differential equation :微分方程partial :偏微分方程Differentiation :求导法implicit :隐求导法partial :偏微分法term by term :逐项求导法Directional derivatives :方向导数Discontinuity :不连续性Disk method :圆盘法Distance :距离Divergence :发散Domain :定义域Dot product :点积Double integral :二重积分change of variable in :二重积分之变数变换in polar coordinates :极坐标二重积分C:Calculus :微积分differential :微分学integral :积分学Cartesian coordinates :笛卡儿坐标一般指直角坐标Cartesian coordinates system :笛卡儿坐标系Cauch’s Mean Value Theorem :柯西均值定理Chain Rule :连锁律Change of variables :变数变换Circle :圆Circular cylinder :圆柱Closed interval :封闭区间Coefficient :系数Composition of function :函数之合成Compound interest :复利Concavity :凹性Conchoid :蚌线Cone :圆锥Constant function :常数函数Constant of integration :积分常数Continuity :连续性at a point :在一点处之连续性of a function :函数之连续性on an interval :在区间之连续性from the left :左连续from the right :右连续Continuous function :连续函数Convergence :收敛interval of :收敛区间radius of :收敛半径Convergent sequence :收敛数列series :收敛级数Coordinate:s:坐标Cartesian :笛卡儿坐标cylindrical :柱面坐标polar :极坐标rectangular :直角坐标spherical :球面坐标Coordinate axes :坐标轴Coordinate planes :坐标平面Cosine function :余弦函数Critical point :临界点Cubic function :三次函数Curve :曲线Cylinder:圆柱Cylindrical Coordinates :圆柱坐标A、B:Absolute convergence :绝对收敛Absolute extreme values :绝对极值Absolute maximum and minimum :绝对极大与极小Absolute value :绝对值Absolute value function :绝对值函数Acceleration :加速度Antiderivative :反导数Approximate integration :近似积分Approximation :逼近法by differentials :用微分逼近linear :线性逼近法by Simpson’s Rule :Simpson法则逼近法by the Trapezoidal Rule :梯形法则逼近法Arbitrary constant :任意常数Arc length :弧长Area :面积under a curve :曲线下方之面积between curves :曲线间之面积in polar coordinates :极坐标表示之面积of a sector of a circle :扇形之面积of a surface of a revolution :旋转曲面之面积Asymptote :渐近线horizontal :水平渐近线slant :斜渐近线vertical :垂直渐近线Average speed :平均速率Average velocity :平均速度Axes, coordinate :坐标轴Axes of ellipse :椭圆之轴Binomial series :二项级数微积分词汇第一章函数与极限Chapter1 Function and Limit集合set元素element子集subset空集empty set并集union交集intersection差集difference of set基本集basic set补集complement set直积direct product笛卡儿积Cartesian product开区间open interval闭区间closed interval半开区间half open interval有限区间finite interval区间的长度length of an interval无限区间infinite interval领域neighborhood领域的中心centre of a neighborhood 领域的半径radius of a neighborhood 左领域left neighborhood右领域right neighborhood映射mappingX到Y的映射mapping of X ontoY 满射surjection单射injection一一映射one—to-one mapping双射bijection算子operator变化transformation函数function逆映射inverse mapping复合映射composite mapping自变量independent variable因变量dependent variable定义域domain函数值value of function函数关系function relation值域range自然定义域natural domain单值函数single valued function多值函数multiple valued function单值分支one—valued branch函数图形graph of a function绝对值函数absolute value符号函数sigh function整数部分integral part阶梯曲线step curve当且仅当if and only if(iff)分段函数piecewise function上界upper bound下界lower bound有界boundedness无界unbounded函数的单调性monotonicity of a function 单调增加的increasing单调减少的decreasing单调函数monotone function函数的奇偶性parity(odevity) of a function 对称symmetry偶函数even function奇函数odd function函数的周期性periodicity of a function周期period反函数inverse function直接函数direct function复合函数composite function中间变量intermediate variable函数的运算operation of function基本初等函数basic elementary function 初等函数elementary function幂函数power function指数函数exponential function对数函数logarithmic function三角函数trigonometric function反三角函数inverse trigonometric function 常数函数constant function双曲函数hyperbolic function双曲正弦hyperbolic sine双曲余弦hyperbolic cosine双曲正切hyperbolic tangent反双曲正弦inverse hyperbolic sine反双曲余弦inverse hyperbolic cosine反双曲正切inverse hyperbolic tangent极限limit数列sequence of number收敛convergence收敛于a converge to a发散divergent极限的唯一性uniqueness of limits收敛数列的有界性boundedness of a convergent sequence子列subsequence函数的极限limits of functions函数当x趋于x0时的极限limit of functions as x approaches x0左极限left limit右极限right limit单侧极限one—sided limits水平渐近线horizontal asymptote无穷小infinitesimal无穷大infinity铅直渐近线vertical asymptote夹逼准则squeeze rule单调数列monotonic sequence高阶无穷小infinitesimal of higher order低阶无穷小infinitesimal of lower order同阶无穷小infinitesimal of the same order作者:新少年特工2007-10-8 18:37 回复此发言—-—--—-—-—-————-—-———-—--——--——----———---——---———————————————---——--———--———----2 高等数学—翻译等阶无穷小equivalent infinitesimal函数的连续性continuity of a function增量increment函数在x0连续the function is continuous at x0左连续left continuous右连续right continuous区间上的连续函数continuous function函数在该区间上连续function is continuous on an interval不连续点discontinuity point第一类间断点discontinuity point of the first kind第二类间断点discontinuity point of the second kind初等函数的连续性continuity of the elementary functions定义区间defined interval最大值global maximum value (absolute maximum)最小值global minimum value (absolute minimum)零点定理the zero point theorem介值定理intermediate value theorem第二章导数与微分Chapter2 Derivative and Differential速度velocity匀速运动uniform motion平均速度average velocity瞬时速度instantaneous velocity圆的切线tangent line of a circle切线tangent line切线的斜率slope of the tangent line位置函数position function导数derivative可导derivable函数的变化率问题problem of the change rate of a function导函数derived function左导数left—hand derivative右导数right—hand derivative单侧导数one—sided derivatives在闭区间【a,b】上可导is derivable on the closed interval [a,b]切线方程tangent equation角速度angular velocity成本函数cost function边际成本marginal cost链式法则chain rule隐函数implicit function显函数explicit function二阶函数second derivative三阶导数third derivative高阶导数nth derivative莱布尼茨公式Leibniz formula对数求导法log— derivative参数方程parametric equation相关变化率correlative change rata微分differential可微的differentiable函数的微分differential of function自变量的微分differential of independent variable微商differential quotient间接测量误差indirect measurement error绝对误差absolute error相对误差relative error第三章微分中值定理与导数的应用Chapter3 MeanValue Theorem of Differentials and the Application of Derivatives罗马定理Rolle’s theorem费马引理Fermat's lemma拉格朗日中值定理Lagrange’s mean value theorem驻点stationary point稳定点stable point临界点critical point辅助函数auxiliary function拉格朗日中值公式Lagrange's mean value formula柯西中值定理Cauchy’s mean value theorem洛必达法则L’Hospital's Rule0/0型不定式indeterminate form of type 0/0不定式indeterminate form泰勒中值定理Taylor’s mean value theorem泰勒公式Taylor formula余项remainder term拉格朗日余项Lagrange remainder term麦克劳林公式Maclaurin’s formula佩亚诺公式Peano remainder term凹凸性concavity凹向上的concave upward, cancave up凹向下的,向上凸的concave downward’ concave down 拐点inflection point函数的极值extremum of function极大值local(relative)maximum最大值global(absolute) mximum极小值local(relative) minimum最小值global(absolute) minimum目标函数objective function曲率curvature弧微分arc differential平均曲率average curvature曲率园circle of curvature曲率中心center of curvature曲率半径radius of curvature渐屈线evolute渐伸线involute根的隔离isolation of root隔离区间isolation interval切线法tangent line method第四章不定积分Chapter4 Indefinite Integrals原函数primitive function(antiderivative)积分号sign of integration被积函数integrand积分变量integral variable积分曲线integral curve积分表table of integrals换元积分法integration by substitution分部积分法integration by parts分部积分公式formula of integration by parts有理函数rational function真分式proper fraction假分式improper fraction第五章定积分Chapter5 Definite Integrals曲边梯形trapezoid with曲边curve edge窄矩形narrow rectangle曲边梯形的面积area of trapezoid with curved edge积分下限lower limit of integral积分上限upper limit of integral积分区间integral interval分割partition积分和integral sum可积integrable矩形法rectangle method积分中值定理mean value theorem of integrals函数在区间上的平均值average value of a function on an integvals 牛顿-莱布尼茨公式Newton—Leibniz formula微积分基本公式fundamental formula of calculus换元公式formula for integration by substitution递推公式recurrence formula反常积分improper integral反常积分发散the improper integral is divergent反常积分收敛the improper integral is convergent无穷限的反常积分improper integral on an infinite interval无界函数的反常积分improper integral of unbounded functions绝对收敛absolutely convergent第六章定积分的应用Chapter6 Applications of the Definite Integrals元素法the element method面积元素element of area平面图形的面积area of a luane figure直角坐标又称“笛卡儿坐标(Cartesian coordinates)”极坐标polar coordinates抛物线parabola椭圆ellipse旋转体的面积volume of a solid of rotation旋转椭球体ellipsoid of revolution,ellipsoid of rotation曲线的弧长arc length of acurve可求长的rectifiable光滑smooth功work水压力water pressure引力gravitation变力variable force第七章空间解析几何与向量代数Chapter7 Space Analytic Geometry and Vector Algebra向量vector自由向量free vector单位向量unit vector零向量zero vector相等equal平行parallel向量的线性运算linear poeration of vector三角法则triangle rule平行四边形法则parallelogram rule交换律commutative law结合律associative law负向量negative vector差difference分配律distributive law空间直角坐标系space rectangular coordinates坐标面coordinate plane卦限octant向量的模modulus of vector向量a与b的夹角angle between vector a and b方向余弦direction cosine方向角direction angle向量在轴上的投影projection of a vector onto an axis数量积,外积,叉积scalar product,dot product,inner product 曲面方程equation for a surface球面sphere旋转曲面surface of revolution母线generating line轴axis圆锥面cone顶点vertex旋转单叶双曲面revolution hyperboloids of one sheet旋转双叶双曲面revolution hyperboloids of two sheets柱面cylindrical surface ,cylinder圆柱面cylindrical surface准线directrix抛物柱面parabolic cylinder二次曲面quadric surface椭圆锥面dlliptic cone椭球面ellipsoid单叶双曲面hyperboloid of one sheet双叶双曲面hyperboloid of two sheets旋转椭球面ellipsoid of revolution椭圆抛物面elliptic paraboloid旋转抛物面paraboloid of revolution双曲抛物面hyperbolic paraboloid马鞍面saddle surface椭圆柱面elliptic cylinder双曲柱面hyperbolic cylinder抛物柱面parabolic cylinder空间曲线space curve空间曲线的一般方程general form equations of a space curve空间曲线的参数方程parametric equations of a space curve螺转线spiral螺矩pitch投影柱面projecting cylinder投影projection平面的点法式方程pointnorm form eqyation of a plane法向量normal vector平面的一般方程general form equation of a plane两平面的夹角angle between two planes点到平面的距离distance from a point to a plane空间直线的一般方程general equation of a line in space方向向量direction vector直线的点向式方程pointdirection form equations of a line方向数direction number直线的参数方程parametric equations of a line两直线的夹角angle between two lines垂直perpendicular直线与平面的夹角angle between a line and a planes平面束pencil of planes平面束的方程equation of a pencil of planes行列式determinant系数行列式coefficient determinant第八章多元函数微分法及其应用Chapter8 Differentiation of Functions of Several Variables and Its Application 一元函数function of one variable多元函数function of several variables内点interior point外点exterior point边界点frontier point,boundary point聚点point of accumulation开集openset闭集closed set连通集connected set开区域open region闭区域closed region有界集bounded set无界集unbounded setn维空间n-dimentional space二重极限double limit多元函数的连续性continuity of function of seveal连续函数continuous function不连续点discontinuity point一致连续uniformly continuous偏导数partial derivative对自变量x的偏导数partial derivative with respect to independent variable x 高阶偏导数partial derivative of higher order二阶偏导数second order partial derivative混合偏导数hybrid partial derivative全微分total differential偏增量oartial increment偏微分partial differential全增量total increment可微分differentiable必要条件necessary condition充分条件sufficient condition叠加原理superpostition principle全导数total derivative中间变量intermediate variable隐函数存在定理theorem of the existence of implicit function曲线的切向量tangent vector of a curve法平面normal plane向量方程vector equation向量值函数vector-valued function切平面tangent plane法线normal line方向导数directional derivative梯度gradient数量场scalar field梯度场gradient field向量场vector field势场potential field引力场gravitational field引力势gravitational potential曲面在一点的切平面tangent plane to a surface at a point曲线在一点的法线normal line to a surface at a point无条件极值unconditional extreme values条件极值conditional extreme values拉格朗日乘数法Lagrange multiplier method拉格朗日乘子Lagrange multiplier经验公式empirical formula最小二乘法method of least squares均方误差mean square error第九章重积分Chapter9 Multiple Integrals二重积分double integral可加性additivity累次积分iterated integral体积元素volume element三重积分triple integral直角坐标系中的体积元素volume element in rectangular coordinate system 柱面坐标cylindrical coordinates柱面坐标系中的体积元素volume element in cylindrical coordinate system 球面坐标spherical coordinates球面坐标系中的体积元素volume element in spherical coordinate system 反常二重积分improper double integral曲面的面积area of a surface质心centre of mass静矩static moment密度density形心centroid转动惯量moment of inertia参变量parametric variable第十章曲线积分与曲面积分Chapter10 Line(Curve)Integrals and Surface Integrals对弧长的曲线积分line integrals with respect to arc hength第一类曲线积分line integrals of the first type对坐标的曲线积分line integrals with respect to x,y,and z第二类曲线积分line integrals of the second type有向曲线弧directed arc单连通区域simple connected region复连通区域complex connected region格林公式Green formula第一类曲面积分surface integrals of the first type对面的曲面积分surface integrals with respect to area有向曲面directed surface对坐标的曲面积分surface integrals with respect to coordinate elements第二类曲面积分surface integrals of the second type有向曲面元element of directed surface高斯公式gauss formula拉普拉斯算子Laplace operator格林第一公式Green's first formula通量flux散度divergence斯托克斯公式Stokes formula环流量circulation旋度rotation,curl第十一章无穷级数Chapter11 Infinite Series一般项general term部分和partial sum余项remainder term等比级数geometric series几何级数geometric series公比common ratio调和级数harmonic series柯西收敛准则Cauchy convergence criteria,Cauchy criteria for convergence 正项级数series of positive terms达朗贝尔判别法D'Alembert test柯西判别法Cauchy test交错级数alternating series绝对收敛absolutely convergent条件收敛conditionally convergent柯西乘积Cauchy product函数项级数series of functions发散点point of divergence收敛点point of convergence收敛域convergence domain和函数sum function幂级数power series幂级数的系数coeffcients of power series阿贝尔定理Abel Theorem收敛半径radius of convergence收敛区间interval of convergence泰勒级数Taylor series麦克劳林级数Maclaurin series二项展开式binomial expansion近似计算approximate calculation舍入误差round-off error,rounding error欧拉公式Euler's formula魏尔斯特拉丝判别法Weierstrass test三角级数trigonometric series振幅amplitude角频率angular frequency初相initial phase矩形波square wave谐波分析harmonic analysis直流分量direct component基波fundamental wave二次谐波second harmonic三角函数系trigonometric function system傅立叶系数Fourier coefficient傅立叶级数Forrier series周期延拓periodic prolongation正弦级数sine series余弦级数cosine series奇延拓odd prolongation偶延拓even prolongation傅立叶级数的复数形式complex form of Fourier series第十二章微分方程Chapter12 Differential Equation解微分方程solve a dirrerential equation常微分方程ordinary differential equation偏微分方程partial differential equation,PDE微分方程的阶order of a differential equation微分方程的解solution of a differential equation微分方程的通解general solution of a differential equation初始条件initial condition微分方程的特解particular solution of a differential equation初值问题initial value problem微分方程的积分曲线integral curve of a differential equation 可分离变量的微分方程variable separable differential equation 隐式解implicit solution隐式通解inplicit general solution衰变系数decay coefficient衰变decay齐次方程homogeneous equation一阶线性方程linear differential equation of first order非齐次non-homogeneous齐次线性方程homogeneous linear equation非齐次线性方程non-homogeneous linear equation常数变易法method of variation of constant暂态电流transient stata current稳态电流steady state current伯努利方程Bernoulli equation全微分方程total differential equation积分因子integrating factor高阶微分方程differential equation of higher order悬链线catenary高阶线性微分方程linera differential equation of higher order自由振动的微分方程differential equation of free vibration强迫振动的微分方程differential equation of forced oscillation串联电路的振荡方程oscillation equation of series circuit二阶线性微分方程second order linera differential equation线性相关linearly dependence线性无关linearly independce二阶常系数齐次线性微分方程second order homogeneour linear differential equation with constant coefficient二阶变系数齐次线性微分方程second order homogeneous linear differential equation with variable coefficient特征方程characteristic equation无阻尼自由振动的微分方程differential equation of free vibration with zero damping固有频率natural frequency简谐振动simple harmonic oscillation,simple harmonic vibration微分算子differential operator待定系数法method of undetermined coefficient共振现象resonance phenomenon欧拉方程Euler equation幂级数解法power series solution数值解法numerial solution勒让德方程Legendre equation微分方程组system of differential equations常系数线性微分方程组system of linera differential equations with constant coefficientV、X、Z:Value of function :函数值Variable :变数Vector :向量Velocity :速度Vertical asymptote :垂直渐近线Volume :体积X—axis :x轴x-coordinate :x坐标x—intercept :x截距Zero vector :函数的零点Zeros of a polynomial :多项式的零点T:Tangent function :正切函数Tangent line :切线Tangent plane :切平面Tangent vector :切向量Total differential :全微分Trigonometric function :三角函数Trigonometric integrals :三角积分Trigonometric substitutions :三角代换法Tripe integrals :三重积分S:Saddle point :鞍点Scalar :纯量Secant line :割线Second derivative :二阶导数Second Derivative Test :二阶导数试验法Second partial derivative :二阶偏导数Sector :扇形Sequence :数列Series :级数Set :集合Shell method :剥壳法Sine function :正弦函数Singularity :奇点Slant asymptote :斜渐近线Slope :斜率Slope-intercept equation of a line :直线的斜截式Smooth curve :平滑曲线Smooth surface :平滑曲面Solid of revolution :旋转体Space :空间Speed :速率Spherical coordinates :球面坐标Squeeze Theorem :夹挤定理Step function :阶梯函数Strictly decreasing :严格递减Strictly increasing :严格递增Sum :和Surface :曲面Surface integral :面积分Surface of revolution :旋转曲面Symmetry :对称R:Radius of convergence :收敛半径Range of a function :函数的值域Rate of change :变化率Rational function :有理函数Rationalizing substitution :有理代换法Rational number :有理数Real number :实数Rectangular coordinates :直角坐标Rectangular coordinate system :直角坐标系Relative maximum and minimum :相对极大值与极小值Revenue function :收入函数Revolution , solid of :旋转体Revolution , surface of :旋转曲面Riemann Sum :黎曼和Riemannian geometry :黎曼几何Right-hand derivative :右导数Right-hand limit :右极限Root :根P、Q:Parabola :拋物线Parabolic cylinder :抛物柱面Paraboloid :抛物面Parallelepiped :平行六面体Parallel lines :并行线Parameter :参数Partial derivative :偏导数Partial differential equation :偏微分方程Partial fractions :部分分式Partial integration :部分积分Partiton :分割Period :周期Periodic function :周期函数Perpendicular lines :垂直线Piecewise defined function :分段定义函数Plane :平面Point of inflection :反曲点Polar axis :极轴Polar coordinate :极坐标Polar equation :极方程式Pole :极点Polynomial :多项式Positive angle :正角Point-slope form :点斜式Power function :幂函数Product :积Quadrant :象限Quotient Law of limit :极限的商定律Quotient Rule :商定律M、N、O:Maximum and minimum values :极大与极小值Mean Value Theorem :均值定理Multiple integrals :重积分Multiplier :乘子Natural exponential function :自然指数函数Natural logarithm function :自然对数函数Natural number :自然数Normal line :法线Normal vector :法向量Number :数Octant :卦限Odd function :奇函数One-sided limit :单边极限Open interval :开区间Optimization problems :最佳化问题Order :阶Ordinary differential equation :常微分方程Origin :原点Orthogonal :正交的L:Laplace transform :Leplace 变换Law of Cosines :余弦定理Least upper bound :最小上界Left-hand derivative :左导数Left-hand limit :左极限Lemniscate :双钮线Length :长度Level curve :等高线L'Hospital's rule :洛必达法则Limacon :蚶线Limit :极限Linear approximation:线性近似Linear equation :线性方程式Linear function :线性函数Linearity :线性Linearization :线性化Line in the plane :平面上之直线Line in space :空间之直线Lobachevski geometry :罗巴切夫斯基几何Local extremum :局部极值Local maximum and minimum :局部极大值与极小值Logarithm :对数Logarithmic function :对数函数I:Implicit differentiation :隐求导法Implicit function :隐函数Improper integral :瑕积分Increasing/Decreasing Test :递增或递减试验法Increment :增量Increasing Function :增函数Indefinite integral :不定积分Independent variable :自变数Indeterminate from :不定型Inequality :不等式Infinite point :无穷极限Infinite series :无穷级数Inflection point :反曲点Instantaneous velocity :瞬时速度Integer :整数Integral :积分Integrand :被积分式Integration :积分Integration by part :分部积分法Intercepts :截距Intermediate value of Theorem :中间值定理Interval :区间Inverse function :反函数Inverse trigonometric function :反三角函数Iterated integral :逐次积分H:Higher mathematics 高等数学/高数E、F、G、H:Ellipse :椭圆Ellipsoid :椭圆体Epicycloid :外摆线Equation :方程式Even function :偶函数Expected Valued :期望值Exponential Function :指数函数Exponents , laws of :指数率Extreme value :极值Extreme Value Theorem :极值定理Factorial :阶乘First Derivative Test :一阶导数试验法First octant :第一卦限Focus :焦点Fractions :分式Function :函数Fundamental Theorem of Calculus :微积分基本定理Geometric series :几何级数Gradient :梯度Graph :图形Green Formula :格林公式Half-angle formulas :半角公式Harmonic series :调和级数Helix :螺旋线Higher Derivative :高阶导数Horizontal asymptote :水平渐近线Horizontal line :水平线Hyperbola :双曲线Hyper boloid :双曲面D:Decreasing function :递减函数Decreasing sequence :递减数列Definite integral :定积分Degree of a polynomial :多项式之次数Density :密度Derivative :导数of a composite function :复合函数之导数of a constant function :常数函数之导数directional :方向导数domain of :导数之定义域of exponential function :指数函数之导数higher :高阶导数partial :偏导数of a power function :幂函数之导数of a power series :羃级数之导数of a product :积之导数of a quotient :商之导数as a rate of change :导数当作变率right-hand :右导数second :二阶导数as the slope of a tangent :导数看成切线之斜率Determinant :行列式Differentiable function :可导函数Differential :微分Differential equation :微分方程partial :偏微分方程Differentiation :求导法implicit :隐求导法partial :偏微分法term by term :逐项求导法Directional derivatives :方向导数Discontinuity :不连续性Disk method :圆盘法Distance :距离Divergence :发散Domain :定义域Dot product :点积Double integral :二重积分。
高等数学-微积分第1章(英文讲稿)

C alc u lus (Fifth Edition)高等数学- Calculus微积分(双语讲稿)Chapter 1 Functions and Models1.1 Four ways to represent a function1.1.1 ☆Definition(1-1) function: A function f is a rule that assigns to each element x in a set A exactly one element, called f(x), in a set B. see Fig.2 and Fig.3Conceptions: domain; range (See fig. 6 p13); independent variable; dependent variable. Four possible ways to represent a function: 1)Verbally语言描述(by a description in words); 2) Numerically数据表述(by a table of values); 3) Visually 视觉图形描述(by a graph);4)Algebraically 代数描述(by an explicit formula).1.1.2 A question about a Curve represent a function and can’t represent a functionThe way ( The vertical line test ) : A curve in the xy-plane is the graph of a function of x if and only if no vertical line intersects the curve more than once. See Fig.17 p 171.1.3 ☆Piecewise defined functions (分段定义的函数)Example7 (P18)1-x if x ≤1f(x)=﹛x2if x>1Evaluate f(0),f(1),f(2) and sketch the graph.Solution:1.1.4 About absolute value (分段定义的函数)⑴∣x∣≥0;⑵∣x∣≤0Example8 (P19)Sketch the graph of the absolute value function f(x)=∣x∣.Solution:1.1.5☆☆Symmetry ,(对称) Even functions and Odd functions (偶函数和奇函数)⑴Symmetry See Fig.23 and Fig.24⑵①Even functions: If a function f satisfies f(-x)=f(x) for every number x in its domain,then f is call an even function. Example f(x)=x2 is even function because: f(-x)= (-x)2=x2=f(x)②Odd functions: If a function f satisfies f(-x)=-f(x) for every number x in its domain,thenf is call an odd function. Example f(x)=x3 is even function because: f(-x)=(-x)3=-x3=-f(x)③Neither even nor odd functions:1.1.6☆☆Increasing and decreasing function (增函数和减函数)⑴Definition(1-2) increasing and decreasing function:① A function f is called increasing on an interval I if f(x1)<f(x2) whenever x1<x2 in I. ①A function f is called decreasing on an interval I if f(x1)>f(x2) whenever x1<x2 in I.See Fig.26. and Fig.27. p211.2 Mathematical models: a catalog of essential functions p251.2.1 A mathematical model p25A mathematical model is a mathematical description of a real-world phenomenon such as the size of a population, the demand for a product, the speed of a falling object, the concentration of a product in a chemical reaction, the life expectancy of a person at birth, or the cost of emission reduction.1.2.2 Linear models and Linear function P261.2.3 Polynomial P27A function f is called a polynomial ifP(x) =a n x n+a n-1x n-1+…+a2x2+a1x+a0Where n is a nonnegative integer and the numbers a0,a1,a2,…,a n-1,a n are constants called the coefficients of the polynomial. The domain of any polynomial is R=(-∞,+∞).if the leading coefficient a n≠0, then the degree of the polynomial is n. For example, the function P(x) =5x6+2x5-x4+3x-9⑴Quadratic function example: P(x) =5x2+2x-3 二次函数(方程)⑵Cubic function example: P(x) =6x3+3x2-1 三次函数(方程)1.2.4Power functions幂函数P30A function of the form f(x) =x a,Where a is a constant, is called a power function. We consider several cases:⑴a=n where n is a positive integer ,(n=1,2,3,…,)⑵a=1/n where n is a positive integer,(n=1,2,3,…,) The function f(x) =x1/n⑶a=n-1 the graph of the reciprocal function f(x) =x-1 反比函数1.2.5Rational function有理函数P 32A rational function f is a ratio of two polynomials:f(x)=P(x) /Q(x)1.2.6Algebraic function代数函数P32A function f is called algebraic function if it can be constructed using algebraic operations ( such as addition,subtraction,multiplication,division,and taking roots) starting with polynomials. Any rational function is automatically an algebraic function. Examples: P 321.2.7Trigonometric functions 三角函数P33⑴f(x)=sin x⑵f(x)=cos x⑶f(x)=tan x=sin x / cos x1.2.8Exponential function 指数函数P34The exponential functions are the functions the form f(x) =a x Where the base a is a positive constant.1.2.9Transcendental functions 超越函数P35These are functions that are not a algebraic. The set of transcendental functions includes the trigonometric,inverse trigonometric,exponential,and logarithmic functions,but it also includes a vast number of other functions that have never been named. In Chapter 11 we will study transcendental functions that are defined as sums of infinite series.1.2 Exercises P 35-381.3 New functions from old functions1.3.1 Transformations of functions P38⑴Vertical and Horizontal shifts (See Fig.1 p39)①y=f(x)+c,(c>0)shift the graph of y=f(x) a distance c units upward.②y=f(x)-c,(c>0)shift the graph of y=f(x) a distance c units downward.③y=f(x+c),(c>0)shift the graph of y=f(x) a distance c units to the left.④y=f(x-c),(c>0)shift the graph of y=f(x) a distance c units to the right.⑵ V ertical and Horizontal Stretching and Reflecting (See Fig.2 p39)①y=c f(x),(c>1)stretch the graph of y=f(x) vertically by a factor of c②y=(1/c) f(x),(c>1)compress the graph of y=f(x) vertically by a factor of c③y=f(x/c),(c>1)stretch the graph of y=f(x) horizontally by a factor of c.④y=f(c x),(c>1)compress the graph of y=f(x) horizontally by a factor of c.⑤y=-f(x),reflect the graph of y=f(x) about the x-axis⑥y=f(-x),reflect the graph of y=f(x) about the y-axisExamples1: (See Fig.3 p39)y=f( x) =cos x,y=f( x) =2cos x,y=f( x) =(1/2)cos x,y=f( x) =cos(x/2),y=f( x) =cos2xExamples2: (See Fig.4 p40)Given the graph y=f( x) =( x)1/2,use transformations to graph y=f( x) =( x)1/2-2,y=f( x) =(x-2)1/2,y=f( x) =-( x)1/2,y=f( x) =2 ( x)1/2,y=f( x) =(-x)1/21.3.2 Combinations of functions (代数组合函数)P42Algebra of functions: Two functions (or more) f and g through the way such as add, subtract, multiply and divide to combined a new function called Combination of function.☆Definition(1-2) Combination function: Let f and g be functions with domains A and B. The functions f±g,f g and f /g are defined as follows: (特别注意符号(f±g)( x) 定义的含义)①(f±g)( x)=f(x)±g( x),domain =A∩B②(f g)( x)=f(x) g( x),domain =A∩ B③(f /g)( x)=f(x) /g( x),domain =A∩ B and g( x)≠0Example 6 If f( x) =( x)1/2,and g( x)=(4-x2)1/2,find functions y=f(x)+g( x),y=f(x)-g( x),y=f(x)g( x),and y=f(x) /g( x)Solution: The domain of f( x) =( x)1/2 is [0,+∞),The domain of g( x) =(4-x2)1/2 is interval [-2,2],The intersection of the domains of f(x) and g( x) is[0,+∞)∩[-2,2]=[0,2]Thus,according to the definitions, we have(f+g)( x)=( x)1/2+(4-x2)1/2,domain [0,2](f-g)( x)=( x)1/2-(4-x2)1/2,domain [0,2](f g)( x)=f(x) g( x) =( x)1/2(4-x2)1/2=(4 x-x3)1/2domain [0,2](f /g)( x)=f(x)/g( x)=( x)1/2/(4-x2)1/2=[ x/(4-x2)]1/2 domain [0,2)1.3.3☆☆Composition of functions (复合函数)P45☆Definition(1-3) Composition function: Given two functions f and g the composite function f⊙g (also called the composition of f and g ) is defined by(f⊙g)( x)=f( g( x)) (特别注意符号(f⊙g)( x) 定义的含义)The domain of f⊙g is the set of all x in the domain of g such that g(x) is in the domain of f . In other words, (f⊙g)(x) is defined whenever both g(x) and f (g (x)) are defined. See Fig.13 p 44 Example7 If f (g)=( g)1/2 and g(x)=(4-x3)1/2find composite functions f⊙g and g⊙f Solution We have(f⊙g)(x)=f (g (x) ) =( g)1/2=((4-x3)1/2)1/2(g⊙f)(x)=g (f (x) )=(4-x3)1/2=[4-((x)1/2)3]1/2=[4-(x)3/2]1/2Example8 If f (x)=( x)1/2 and g(x)=(2-x)1/2find composite function s①f⊙g ②g⊙f ③f⊙f④g⊙gSolution We have①f⊙g=(f⊙g)(x)=f (g (x) )=f((2-x)1/2)=((2-x)1/2)1/2=(2-x)1/4The domain of (f⊙g)(x) is 2-x≥0 that is x ≤2 {x ︳x ≤2 }=(-∞,2]②g⊙f=(g⊙f)(x)=g (f (x) )=g (( x)1/2 )=(2-( x)1/2)1/2The domain of (g⊙f)(x) is x≥0 and 2-( x)1/2x ≥0 ,that is ( x)1/2≤2 ,or x ≤ 4 ,so the domain of g⊙f is the closed interval[0,4]③f⊙f=(f⊙f)(x)=f (f(x) )=f((x)1/2)=((x)1/2)1/2=(x)1/4The domain of (f⊙f)(x) is [0,∞)④g⊙g=(g⊙g)(x)=g (g(x) )=g ((2-x)1/2 )=(2-(2-x)1/2)1/2The domain of (g⊙g)(x) is x-2≥0 and 2-(2-x)1/2≥0 ,that is x ≤2 and x ≥-2,so the domain of g⊙g is the closed interval[-2,2]Notice: g⊙f⊙h=f (g(h(x)))Example9Example10 Given F (x)=cos2( x+9),find functions f,g,and h such that F (x)=f⊙g⊙h Solution Since F (x)=[cos ( x+9)] 2,that is h (x)=x+9 g(x)=cos x f (x)=x2Exercise P 45-481.4 Graphing calculators and computers P481.5 Exponential functions⑴An exponential function is a function of the formf (x)=a x See Fig.3 P56 and Fig.4Exponential functions increasing and decreasing (单调性讨论)⑵Lows of exponents If a and b are positive numbers and x and y are any real numbers. Then1) a x+y=a x a y2) a x-y=a x / a y3) (a x)y=a xy4) (ab)x+y=a x b x⑶about the number e f (x)=e x See Fig. 14,15 P61Some of the formulas of calculus will be greatly simplified if we choose the base a .Exercises P 62-631.6 Inverse functions and logarithms1.6.1 Definition(1-4) one-to-one function: A function f is called a one-to-one function if it never takes on the same value twice;that is,f (x1)≠f (x2),whenever x1≠x2( 注解:不同的自变量一定有不同的函数值,不同的自变量有相同的函数值则不是一一对应函数) Example: f (x)=x3is one-to-one function.f (x)=x2 is not one-to-one function, See Fig.2,3,4☆☆Definition(1-5) Inverse function:Let f be a one-to-one function with domain A and range B. Then its inverse function f-1(y)has domain B and range A and is defined byf-1(y)=x f (x)=y for any y in Bdomain of f-1=range of frange of f-1=domain of f( 注解:it says : if f maps x into y, then f-1maps y back into x . Caution: If f were not one-to-one function,then f-1 would not be uniquely defined. )Caution: Do not mistake the-1 in f-1for an exponent. Thus f-1(x)=1/ f(x) !!!Because the letter x is traditionally used as the independent variable, so when we concentrate on f-1(y) rather than on f-1(y), we usually reverse the roles of x and y in Definition (1-5) and write as f-1(x)=y f (x)=yWe get the following cancellation equations:f-1( f(x))=x for every x in Af (f-1(x))=x for every x in B See Fig.7 P66Example 4 Find the inverse function of f(x)=x3+6Solution We first writef(x)=y=x3+6Then we solve this equation for x:x3=y-6x=(y-6)1/3Finally, we interchange x and y:y=(x-6)1/3That is, the inverse function is f-1(x)=(x-6)1/3( 注解:The graph of f-1 is obtained by reflecting the graph of f about the line y=x. ) See Fig.9、8 1.6.2 Logarithmic functionIf a>0 and a≠1,the exponential function f (x)=a x is either increasing or decreasing and so it is one-to-one function by the Horizontal Line Test. It therefore has an inverse function f-1,which is called the logarithmic function with base a and is denoted log a,If we use the formulation of an inverse function given by (See Fig.3 P56)f-1(x)=y f (x)=yThen we havelogx=y a y=xThe logarithmic function log a x=y has domain (0,∞) and range R.Usefully equations:①log a(a x)=x for every x∈R②a log ax=x for every x>01.6.3 ☆Lows of logarithms :If x and y are positive numbers, then①log a(xy)=log a x+log a y②log a(x/y)=log a x-log a y③log a(x)r=r log a x where r is any real number1.6.4 Natural logarithmsNatural logarithm isl og e x=ln x =ythat is①ln x =y e y=x② ln(e x)=x x∈R③e ln x=x x>0 ln e=1Example 8 Solve the equation e5-3x=10Solution We take natural logarithms of both sides of the equation and use ②、③ln (e5-3x)=ln10∴5-3x=ln10x=(5-ln10)/3Example 9 Express ln a+(ln b)/2 as a single logarithm.Solution Using laws of logarithms we have:ln a+(ln b)/2=ln a+ln b1/2=ln(ab1/2)1.6.5 ☆Change of Base formula For any positive number a (a≠1), we havel og a x=ln x/ ln a1.6.6 Inverse trigonometric functions⑴Inverse sine function or Arcsine functionsin-1x=y sin y=x and -π/2≤y≤π / 2,-1≤x≤1 See Fig.18、20 P72Example13 ① sin-1 (1/2) or arcsin(1/2) ② tan(arcsin1/3)Solution①∵sin (π/6)=1/2,π/6 lies between -π/2 and π / 2,∴sin-1 (1/2)=π/6② Let θ=arcsin1/3,so sinθ=1/3tan(arcsin1/3)=tanθ= s inθ/cosθ= (1/3)/(1-s in2θ)1/2=1/(8)1/2Usefully equations:①sin-1(sin x)=x for -π/2≤x≤π / 2②sin (sin-1x)=x for -1≤x≤1⑵Inverse cosine function or Arccosine functioncos-1x=y cos y=x and 0 ≤y≤π,-1≤x≤1 See Fig.21、22 P73Usefully equations:①cos-1(cos x)=x for 0 ≤x≤π②cos (cos-1x)=x for -1≤x≤1⑶Inverse Tangent function or Arctangent functiontan-1x=y tan y=x and -π/2<y<π / 2 ,x∈R See Fig.23 P73、Fig.25 P74Example 14 Simplify the expression cos(ta n-1x).Solution 1 Let y=tan-1 x,Then tan y=x and -π/2<y<π / 2 ,We want find cos y but since tan y is known, it is easier to find sec y first:sec2y=1 +tan2y sec y=(1 +x2 )1/2∴cos(ta n-1x)=cos y =1/ sec y=(1 +x2)-1/2Solution 2∵cos(ta n-1x)=cos y∴cos(ta n-1x)=(1 +x2)-1/2⑷Other Inverse trigonometric functionscsc-1x=y∣x∣≥1csc y=x and y∈(0,π / 2]∪(π,3π / 2]sec-1x=y∣x∣≥1sec y=x and y∈[0,π / 2)∪[π,3π / 2]cot-1x=y x∈R cot y=x and y∈(0,π)Exercises P 74-85Key words and PhrasesCalculus 微积分学Set 集合Variable 变量Domain 定义域Range 值域Arbitrary number 独立变量Independent variable 自变量Dependent variable 因变量Square root 平方根Curve 曲线Interval 区间Interval notation 区间符号Closed interval 闭区间Opened interval 开区间Absolute 绝对值Absolute value 绝对值Symmetry 对称性Represent of a function 函数的表述(描述)Even function 偶函数Odd function 奇函数Increasing Function 增函数Increasing Function 减函数Empirical model 经验模型Essential Function 基本函数Linear function 线性函数Polynomial function 多项式函数Coefficient 系数Degree 阶Quadratic function 二次函数(方程)Cubic function 三次函数(方程)Power functions 幂函数Reciprocal function 反比函数Rational function 有理函数Algebra 代数Algebraic function 代数函数Integer 整数Root function 根式函数(方程)Trigonometric function 三角函数Exponential function 指数函数Inverse function 反函数Logarithm function 对数函数Inverse trigonometric function 反三角函数Natural logarithm function 自然对数函数Chang of base of formula 换底公式Transcendental function 超越函数Transformations of functions 函数的变换Vertical shifts 垂直平移Horizontal shifts 水平平移Stretch 伸张Reflect 反演Combinations of functions 函数的组合Composition of functions 函数的复合Composition function 复合函数Intersection 交集Quotient 商Arithmetic 算数。
第二节·有理函数求导规则
u( x ) 1 ( u( x ) 0) . (cu)( x ) cu( x ) ( c R is a constant), ( x ) 2 u ( x) u
In particular
2
Derivation rules for sum, difference, product and quotient of functions
dy ( x 2 ) (2 x ) (3cos x ) ( x ln x ) dx 2 x 2 x ln 2 3sin x ( x ) ln x x (ln x )
2 x 2 x ln 2 3sin x ln x 2 x x x
2 x 2 x ln 2 3sin x
dy d d 1 2 1 1 2 1 . x 2 . 2 2 dx dx dx x x x
The slope at x = 1 is
dy dx 2 x 1 1 2 x
x 1
1 2. 1.
x 3 3 x 2 2 x . 1 2 3 y x 3 x 2 x . 4 4 x x Then use the Sum and Power Rules:
x 1 x 2 2 x
dy 1 6 6 2 3 4 . x 3 2 x 2 3 x 2 3 4 . dx x x x
By the definition of the derivative, we have
( uv )( x ) lim
y u v . lim v ( x x ) lim u( x ) x 0 x x 0 x x x 0
斯普林格数学研究生教材丛书

斯普林格数学研究生教材丛书《斯普林格数学研究生教材丛书》(Graduate Texts in Mathematics)GTM001《Introduction to Axiomatic Set Theory》Gaisi Takeuti, Wilson M.Zaring GTM002《Measure and Category》John C.Oxtoby(测度和范畴)(2ed.)GTM003《T opological Vector Spaces》H.H.Schaefer, M.P.Wolff(2ed.)GTM004《A Course in Homological Algebra》P.J.Hilton, U.Stammbach(2ed.)(同调代数教程)GTM005《Categories for the Working Mathematician》Saunders Mac Lane(2ed.)GTM006《Projective Planes》Daniel R.Hughes, Fred C.Piper(投射平面)GTM007《A Course in Arithmetic》Jean-Pierre Serre(数论教程)GTM008《Axiomatic set theory》Gaisi Takeuti, Wilson M.Zaring(2ed.)GTM009《Introduction to Lie Algebras and Representation Theory》James E.Humphreys(李代数和表示论导论)GTM010《A Course in Simple-Homotopy Theory》M.M CohenGTM011《Functions of One Complex VariableⅠ》John B.ConwayGTM012《Advanced Mathematical Analysis》Richard Beals GTM013《Rings and Categories of Modules》Frank W.Anderson, Kent R.Fuller(环和模的范畴)(2ed.)GTM014《Stable Mappings and Their Singularities》Martin Golubitsky, Victor Guillemin (稳定映射及其奇点)GTM015《Lectures in Functional Analysis and OperatorTheory》Sterling K.Berberian GTM016《The Structure of Fields》David J.Winter(域结构)GTM017《Random Processes》Murray RosenblattGTM018《Measure Theory》Paul R.Halmos(测度论)GTM019《A Hilbert Space Problem Book》Paul R.Halmos (希尔伯特问题集)GTM020《Fibre Bundles》Dale Husemoller(纤维丛)GTM021《Linear Algebraic Groups》James E.Humphreys (线性代数群)GTM022《An Algebraic Introduction to Mathematical Logic》Donald W.Barnes, John M.MackGTM023《Linear Algebra》Werner H.Greub(线性代数)GTM024《Geometric Functional Analysis and Its Applications》Paul R.HolmesGTM025《Real and Abstract Analysis》Edwin Hewitt, Karl StrombergGTM026《Algebraic Theories》Ernest G.ManesGTM027《General Topology》John L.Kelley(一般拓扑学)GTM028《Commutative Algebra》VolumeⅠOscar Zariski, Pierre Samuel(交换代数)GTM029《Commutative Algebra》VolumeⅡOscar Zariski, Pierre Samuel(交换代数)GTM030《Lectures in Abstract AlgebraⅠ.Basic Concepts》Nathan Jacobson(抽象代数讲义Ⅰ基本概念分册)GTM031《Lectures in Abs tract AlgebraⅡ.Linear Algabra》Nathan.Jacobson(抽象代数讲义Ⅱ线性代数分册)GTM032《Lectures in Abstract AlgebraⅢ.Theory of Fields and Galois Theory》Nathan.Jacobson(抽象代数讲义Ⅲ域和伽罗瓦理论)GTM033《Differential Topology》Morris W.Hirsch(微分拓扑)GTM034《Principles of Random Walk》Frank Spitzer(2ed.)(随机游动原理)GTM035《Several Complex Variables and Banach Algebras》Herbert Alexander, John Wermer(多复变和Banach代数)GTM036《Linear Topological Spaces》John L.Kelley, Isaac Namioka(线性拓扑空间)GTM037《Mathematical Logic》J.Donald Monk(数理逻辑)GTM038《Several Complex Variables》H.Grauert, K.Fritzshe GTM039《An Invitation to C*-Algebras》William Arveson (C*-代数引论)GTM040《Denumerable Markov Chains》John G.Kemeny, /doc/e96250642.htmlurie Snell, Anthony W.KnappGTM041《Modular Functions and Dirichlet Series in Number Theory》Tom M.Apostol (数论中的模函数和Dirichlet序列)GTM042《Linear Representations of Finite Groups》Jean-Pierre Serre(有限群的线性表示)GTM043《Rings of Continuous Functions》Leonard Gillman, Meyer JerisonGTM044《Elementary Algebraic Geometry》Keith KendigGTM045《Probabi lity TheoryⅠ》M.Loève(概率论Ⅰ)(4ed.)GTM046《Probability TheoryⅡ》M.Loève(概率论Ⅱ)(4ed.)GTM047《Geometric Topology in Dimensions 2 and 3》Edwin E.MoiseGTM048《General Relativity for Mathematicians》Rainer.K.Sachs, H.Wu伍鸿熙(为数学家写的广义相对论)GTM049《Linear Geometry》K.W.Gruenberg, A.J.Weir(2ed.)GTM050《Fermat's Last Theorem》Harold M.EdwardsGTM051《A Course in Differential Geometry》WilhelmKlingenberg(微分几何教程)GTM052《Algebraic Geometry》Robin Hartshorne(代数几何)GTM053《 A Course in Mathematical Logic for Mathematicians》Yu.I.Manin(2ed.)GTM054《Combinatorics with Emphasis on the Theory of Graphs》Jack E.Graver, Mark E.WatkinsGTM055《Introduction to Operator TheoryⅠ》Arlen Brown, Carl PearcyGTM056《Algebraic Topology:An Introduction》W.S.MasseyGTM057《Introduction to Knot Theory》Richard.H.Crowell, Ralph.H.FoxGTM058《p-adic Numbers, p-adic Analysis, and Zeta-Functions》Neal Koblitz(p-adic 数、p-adic分析和Z函数)GTM059《Cyclotomic Fields》Serge LangGTM060《Mathematical Methods of Classical Mechanics》V.I.Arnold(经典力学的数学方法)(2ed.)GTM061《Elements of Homotopy Theory》George W.Whitehead(同论论基础)GTM062《Fundamentals of the Theory of Groups》M.I.Kargapolov, Ju.I.Merzljakov GTM063《Modern Graph Theory》Béla BollobásGTM064《Fourier Series:A Modern Introduction》VolumeⅠ(2ed.)R.E.Edwards(傅里叶级数)GTM065《Differential Analysis on Complex Manifolds》Raymond O.Wells, Jr.(3ed.)GTM066《Introduction to Affine Group Schemes》William C.Waterhouse(仿射群概型引论)GTM067《Local Fields》Jean-Pierre Serre(局部域)GTM069《Cyclotomic FieldsⅠandⅡ》Serge LangGTM070《Singular Homology Theory》William S.MasseyGTM071《Riemann Surfaces》Herschel M.Farkas, Irwin Kra (黎曼曲面)GTM072《Classical Topology and Combinatorial Group Theory》John Stillwell(经典拓扑和组合群论)GTM073《Algebra》Thomas W.Hungerford(代数)GTM074《Multiplicative Number Theory》Harold Davenport (乘法数论)(3ed.)GTM075《Basic Theory of Algebraic Groups and Lie Algebras》G.P.HochschildGTM076《Algebraic Geometry:An Introduction to Birational Geometry of Algebraic Varieties》Shigeru Iitaka GTM077《Lectures on the Theory of Algebraic Numbers》Erich HeckeGTM078《A Course in Universal Algebra》Stanley Burris, H.P.Sankappanavar(泛代数教程)GTM079《An Introduction to Ergodic Theory》Peter Walters (遍历性理论引论)GTM080《A Course in_the Theory of Groups》Derek J.S.RobinsonGTM081《Lectures on Riemann Surfaces》Otto ForsterGTM082《Differential Forms in Algebraic Topology》Raoul Bott, Loring W.Tu(代数拓扑中的微分形式)GTM083《Introduction to Cyclotomic Fields》Lawrence C.Washington(割圆域引论)GTM084《A Classical Introduction to Modern Number Theory》Kenneth Ireland, Michael Rosen(现代数论经典引论)GTM085《Fourier Series A Modern Introduction》Volume 1(2ed.)R.E.Edwards GTM086《Introduction to Coding Theory》J.H.van Lint(3ed .)GTM087《Cohomology of Groups》Kenneth S.Brown(上同调群)GTM088《Associative Algebras》Richard S.PierceGTM089《Introduction to Algebraic and Abelian Functions》Serge Lang(代数和交换函数引论)GTM090《An Introduction to Convex Polytopes》Ame BrondstedGTM091《The Geometry of Discrete Groups》Alan F.BeardonGTM092《Sequences and Series in BanachSpaces》Joseph DiestelGTM093《Modern Geometry-Methods and Applications》(PartⅠ.The of geometry Surface s Transformation Groups and Fields)B.A.Dubrovin, A.T.Fomenko, S.P.Novikov (现代几何学方法和应用)GTM094《Foundations of Differentiable Manifolds and Lie Groups》Frank W.Warner(可微流形和李群基础)GTM095《Probability》A.N.Shiryaev(2ed.)GTM096《A Course in Functional Analysis》John B.Conway (泛函分析教程)GTM097《Introduction to Elliptic Curves and Modular Forms》Neal Koblitz(椭圆曲线和模形式引论)GTM098《Representations of Compact Lie Groups》Theodor Bre?cker, Tammo tom DieckGTM099《Finite Reflection Groups》L.C.Grove, C.T.Benson (2ed.)GTM100《Harmonic Analysis on Semigroups》Christensen Berg, Jens Peter Reus Christensen, Paul ResselGTM101《Galois Theory》Harold M.Edwards(伽罗瓦理论)GTM102《Lie Groups, Lie Algebras, and Their Representation》V.S.Varadarajan(李群、李代数及其表示)GTM103《Complex Analysis》Serge LangGTM104《Modern Geometry-Methods and Applications》(PartⅡ.Geometry and Topology of Manifolds)B.A.Dubrovin, A.T.Fomenko, S.P.Novikov(现代几何学方法和应用)GTM105《SL? (R)》Serge Lang(SL? (R)群)GTM106《The Arithmetic of Elliptic Curves》Joseph H.Silverman(椭圆曲线的算术理论)GTM107《Applications of Lie Groups to Differential Equations》Peter J.Olver(李群在微分方程中的应用)GTM108《Holomorphic Functions and Integral Representations in Several Complex Variables》R.Michael Range GTM109《Univalent Functions and Teichmueller Spaces》Lehto OlliGTM110《Algebraic Number Theory》Serge Lang(代数数论)GTM111《Elliptic Curves》Dale Husemoeller(椭圆曲线)GTM112《Elliptic Functions》Serge Lang(椭圆函数)GTM113《Brownian Motion and Stochastic Calculus》Ioannis Karatzas, Steven E.Shreve (布朗运动和随机计算)GTM114《A Course in Number Theory and Cryptography》Neal Koblitz(数论和密码学教程)GTM115《Differential Geometry:Manifolds, Curves, and Surfaces》M.Berger, B.Gostiaux GTM116《Measure and Integral》Volume1 John L.Kelley, T.P.SrinivasanGTM117《Algebraic Groups and Class Fields》Jean-Pierre Serre(代数群和类域)GTM118《Analysis Now》Gert K.Pedersen (现代分析)GTM119《An introduction to Algebraic Topology》Jossph J.Rotman(代数拓扑导论)GTM120《Weakly Differentiable Functions》William P.Ziemer(弱可微函数)GTM121《Cyclotomic Fields》Serge LangGTM122《Theory of Complex Functions》Reinhold RemmertGTM123《Numbers》H.-D.Ebbinghaus, H.Hermes, F.Hirzebruch, M.Koecher, K.Mainzer, J.Neukirch, A.Prestel, R.Remmert(2ed.)GTM124《Modern Geometry-Methods and Applications》(PartⅢ.Introduction to Homology Theory)B.A.Dubrovin, A.T.Fomenko, S.P.Novikov(现代几何学方法和应用)GTM125《Complex Variables:An introduction》Garlos A.Berenstein, Roger Gay GTM126《Linear Algebraic Groups》Armand Borel (线性代数群)GTM127《A Basic Course in Algebraic Topology》William S.Massey(代数拓扑基础教程)GTM128《Partial Differential Equations》Jeffrey RauchGTM129《Representation Theory:A First Course》William Fulton, Joe HarrisGTM130《T ensor Geometry》C.T.J.Dodson, T.Poston(张量几何)GTM131《A First Course in Noncommutative Rings》/doc/e96250642.htmlm(非交换环初级教程)GTM132《Iteration of Rational Functions:Complex Analytic Dynamical Systems》AlanF.Beardon(有理函数的迭代:复解析动力系统)GTM133《Algebraic Geometry:A First Course》Joe Harris (代数几何)GTM134《Coding and Information Theory》Steven Roman GTM135《Advanced Linear Algebra》Steven RomanGTM136《Algebra:An Approach via Module Theory》William A.Adkins, Steven H.WeintraubGTM137《Harmonic Function Theory》Sheldon Axler, Paul Bourdon, Wade Ramey(调和函数理论)GTM138《A Course in Computational Algebraic NumberTheory》Henri Cohen(计算代数数论教程)GTM139《T opology and Geometry》Glen E.BredonGTM140《Optima and Equilibria:An Introduction to Nonlinear Analysis》Jean-Pierre AubinGTM141《A Computational Approach to Commutative Algebra》Gr?bner Bases, Thomas Becker, Volker Weispfenning, Heinz KredelGTM142《Real and Functional Analysis》Serge Lang(3ed.)GTM143《Measure Theory》J.L.DoobGTM144《Noncommutative Algebra》Benson Farb, R.Keith DennisGTM145《Homology Theory:An Introduction to Algebraic Topology》James W.Vick(同调论:代数拓扑简介)GTM146《Computability:A Mathematical Sketchbook》Douglas S.BridgesGTM147《Algebraic K-Theory and Its Applications》Jonathan Rosenberg(代数K理论及其应用)GTM148《An Introduction to the Theory of Groups》Joseph J.Rotman(群论入门)GTM149《Foundations of Hyperbolic Manifolds》John G.Ratcliffe(双曲流形基础)GTM150《Commutative Algebra with a view toward Algebraic Geometry》David EisenbudGTM151《Advanced Topics in the Arithmetic of Elliptic Curves》Joseph H.Silverman(椭圆曲线的算术高级选题)GTM152《Lectures on Polytopes》Günter M.ZieglerGTM153《Algebraic Topology:A First Course》William Fulton(代数拓扑)GTM154《An introduction to Analysis》Arlen Brown, Carl PearcyGTM155《Quantum Groups》Christian Kassel(量子群)GTM156《Classical Descriptive Set Theory》Alexander S.KechrisGTM157《Integration and Probability》Paul MalliavinGTM158《Field theory》Steven Roman(2ed.)GTM159《Functions of One Complex Variable VolⅡ》John B.ConwayGTM160《Differential and Riemannian Manifolds》Serge Lang(微分流形和黎曼流形)GTM161《Polynomials and Polynomial Inequalities》Peter Borwein, Tamás Erdélyi(多项式和多项式不等式)GTM162《Groups and Representations》J.L.Alperin, Rowen B.Bell(群及其表示)GTM163《Permutation Groups》John D.Dixon, Brian Mortime rGTM164《Additive Number Theory:The Classical Bases》Melvyn B.NathansonGTM165《Additive Number Theory:Inverse Problems and the Geometry of Sumsets》Melvyn B.NathansonGTM166《Differential Geometry:Cartan's Generalization of Klein's Erlangen Program》R.W.SharpeGTM167《Field and Galois Theory》Patrick MorandiGTM168《Combinatorial Convexity and Algebraic Geometry》Günter Ewald(组合凸面体和代数几何)GTM169《Matrix Analysis》Rajendra BhatiaGTM170《Sheaf Theory》Glen E.Bredon(2ed.)GTM171《Riemannian Geometry》Peter Petersen(黎曼几何)GTM172《Classical Topics in Complex Function Theory》Reinhold RemmertGTM173《Graph Theory》Reinhard Diestel(图论)(3ed.)GTM174《Foundations of Real and Abstract Analysis》Douglas S.Bridges(实分析和抽象分析基础)GTM175《An Introduction to Knot Theory》W.B.Raymond LickorishGTM176《Riemannian Manifolds:An Introduction to Curvature》John M.LeeGTM177《Analytic Number Theory》Donald J.Newman(解析数论)GTM178《Nonsmooth Analysis and Control Theory》F.H.clarke, Yu.S.Ledyaev, R.J.Stern, P.R.Wolenski(非光滑分析和控制论)GTM179《Banach Algebra Techniques in Operator Theory》Ronald G.Douglas(2ed.)GTM180《A Course on Borel Sets》S.M.Srivastava(Borel 集教程)GTM181《Numerical Analysis》Rainer KressGTM182《Ordinary Differential Equations》Wolfgang Walter GTM183《An introduction to Banach Spaces》Robert E.MegginsonGTM184《Modern Graph Theory》Béla Bollobás(现代图论)GTM185《Using Algebraic Geomety》David A.Cox, John Little, Donal O’Shea(应用代数几何)GTM186《Fourier Analysis on Number Fields》Dinakar Ramakrishnan, Robert J.Valenza GTM187《Moduli of Curves》Joe Harris, Ian Morrison(曲线模)GTM188《Lectures on the Hyperreals:An Introduction to Nonstandard Analysis》Robert GoldblattGTM189《Lectures on Modules and Rings》/doc/e96250642.htmlm(模和环讲义)GTM190《Problems in Algebraic Number Theory》M.Ram Murty, Jody Esmonde(代数数论中的问题)GTM191《Fundamentals of Differential Geometry》Serge Lang(微分几何基础)GTM192《Elements of Functional Analysis》Francis Hirsch, Gilles LacombeGTM193《Advanced Topics in Computational Number Theory》Henri CohenGTM194《One-Parameter Semigroups for Linear Evolution Equations》Klaus-Jochen Engel, Rainer Nagel(线性发展方程的单参数半群)GTM195《Elementary Methods in Number Theory》Melvyn B.Nathanson(数论中的基本方法)GTM196《Basic Homological Algebra》M.Scott OsborneGTM197《The Geometry of Schemes》David Eisenbud, Joe HarrisGTM198《A Course in p-adic Analysis》Alain M.RobertGTM199《Theory of Bergman Spaces》Hakan Hedenmalm, Boris Korenblum, Kehe Zhu(Bergman空间理论)GTM200《An Introduction to Riemann-Finsler Geometry》D.Bao, S.-S.Chern, Z.Shen GTM201《Diophantine Geometry An Introduction》Marc Hindry, Joseph H.Silverman GTM202《Introduction to T opological Manifolds》John M.Lee GTM203《The Symmetric Group》Bruce E.SaganGTM204《Galois Theory》Jean-Pierre EscofierGTM205《Rational Homotopy Theory》Yves Félix, Stephen Halperin, Jean-Claude Thomas(有理同伦论)GTM206《Problems in Analytic Number Theory》M.Ram MurtyGTM207《Algebraic Graph Theory》Chris Godsil, Gordon Royle(代数图论)GTM208《Analysis for Applied Mathematics》Ward Cheney GTM209《A Short Course on Spectral Theory》William Arveson(谱理论简明教程)GTM210《Number Theory in Function Fields》Michael RosenGTM211《Algebra》Serge Lang(代数)GTM212《Lectures on Discrete Geometry》Jiri Matousek (离散几何讲义)GTM213《From Holomorphic Functions to Complex Manifolds》Klaus Fritzsche, Hans Grauert(从正则函数到复流形)GTM214《Partial Differential Equations》Jüergen Jost(偏微分方程)GTM215《Algebraic Functions and Projective Curves》David M.Goldschmidt(代数函数和投影曲线)GTM216《Matrices:Theory and Applications》Denis Serre (矩阵:理论及应用)GTM217《Model Theory An Introduction》David Marker(模型论引论)GTM218《Introduction to Smooth Manifolds》John M.Lee (光滑流形引论)GTM219《The Arithmetic of Hyperbolic 3-Manifolds》Colin Maclachlan, Alan W.Reid GTM220《Smooth Manifolds and Observables》Jet Nestruev(光滑流形和直观)GTM221《Convex Polytopes》Branko GrüenbaumGTM222《Lie Groups, Lie Algebras, and Representations》Brian C.Hall(李群、李代数和表示)GTM223《Fourier Analysis and its Applications》Anders Vretblad(傅立叶分析及其应用)GTM224《Metric Structures in Differential Geometry》Gerard Walschap(微分几何中的度量结构)GTM225《Lie Groups》Daniel Bump(李群)GTM226《Spaces of Holomorphic Functions in the Unit Ball》Kehe Zhu(单位球内的全纯函数空间)GTM227《Combinatorial Commutative Algebra》Ezra Miller, Bernd Sturmfels(组合交换代数)GTM228《A First Course in Modular Forms》Fred Diamond, Jerry Shurman(模形式初级教程)GTM229《The Geometry of Syzygies》David Eisenbud(合冲几何)GTM230《An Introduction to Markov Processes》Daniel W.Stroock(马尔可夫过程引论)GTM231《Combinatorics of Coxeter Groups》Anders Bjr?ner, Francesco Brenti(Coxeter 群的组合学)GTM232《An Introduction to Number Theory》Graham Everest, Thomas Ward(数论入门)GTM233《T opics in Banach Space Theory》Fenando Albiac, Nigel J.Kalton(Banach空间理论选题)GTM234《Analysis and Probability:Wavelets, Signals, Fractals》Palle E.T.Jorgensen(分析与概率)GTM235《Compact Lie Groups》Mark R.Sepanski(紧致李群)GTM236《Bounded Analytic Functions》John B.Garnett(有界解析函数)GTM237《An Introduction to Operators on the Hardy-Hilbert Space》Rubén A.Martínez-Avendano, Peter Rosenthal (哈代-希尔伯特空间算子引论)GTM238《A Course in Enumeration》Martin Aigner(枚举教程)GTM239《Number Theory:VolumeⅠT ools and Diophantine Equations》Henri Cohen GTM240《Number Theory:VolumeⅡAna lytic and Modern T ools》Henri Cohen GTM241《The Arithmetic of Dynamical Systems》Joseph H.SilvermanGTM242《Abstract Algebra》Pierre Antoine Grillet(抽象代数)GTM243《Topological Methods in Group Theory》Ross GeogheganGTM244《Graph Theory》J.A.Bondy, U.S.R.MurtyGTM245《Complex Analysis:In the Spirit of Lipman Bers》Jane P.Gilman, Irwin Kra, Rubi E.RodriguezGTM246《A Course in Commutative Banach Algebras》Eberhard KaniuthGTM247《Braid Groups》Christian Kassel, Vladimir TuraevGTM248《Buildings Theory and Applications》Peter Abramenko, Kenneth S.Brown GTM249《Classical Fourier Analysis》Loukas Grafakos(经典傅里叶分析)GTM250《Modern Fourier Analysis》Loukas Grafakos(现代傅里叶分析)GTM251《The Finite Simple Groups》Robert A.WilsonGTM252《Distributions and Operators》Gerd GrubbGTM253《Elementary Functional Analysis》Barbara D.MacCluerGTM254《Algebraic Function Fields and Codes》Henning StichtenothGTM255《Symmetry Representations and Invariants》Roe Goodman, Nolan R.Wallach GTM256《A Course in Commutative Algebra》Kemper GregorGTM257《Deformation Theory》Robin HartshorneGTM258《Foundation of Optimization》Osman GülerGTM259《Ergodic Theory:with a view towards Number Theory》Manfred Einsiedler, Thomas WardGTM260《Monomial Ideals》Jurgen Herzog, Takayuki Hibi GTM261《Probability and Stochastics》Erhan CinlarGTM262《Essentials of Integration Theory for Analysis》Daniel W.StroockGTM263《Analysis on Fock Spaces》Kehe ZhuGTM264《Functional Analysis, Calculus of Variations and Optimal Control》Francis ClarkeGTM265《Unbounded Self-adjoint Operatorson Hilbert Space》Konrad Schmüdgen GTM266《Calculus Without Derivatives》Jean-Paul PenotGTM267《Quantum Theory for Mathematicians》Brian C.HallGTM268《Geometric Analysis of the Bergman Kernel and Metric》Steven G.Krantz GTM269《Locally Convex Spaces》M.Scott Osborne。
rational函数 -回复

rational函数-回复什么是有理函数(rational函数)?有理函数(rational function)是指由两个多项式的商组成的函数。
其中,被除式(denominator)为非零多项式,而除式(numerator)可以为零多项式。
有理函数可以表示为:f(x) = P(x) / Q(x)其中P(x)和Q(x)都是多项式函数,且Q(x) ≠0。
有理函数在数学中具有重要的地位,因为它们既包含了多项式函数的特点,也包含了分式函数的特点。
首先,让我们了解有理函数的定义和范围。
有理函数定义于除了可能使得其分母为零的一些点之外的所有实数。
因此,在定义有理函数时,我们需排除导致分母为零的输入值。
下一步,我们将讨论有理函数的基本性质。
首先,我们会探讨有理函数的定义域,并确定任何使得分母为零的情况。
这一步骤是非常重要的,因为它可以帮助我们确定函数的可行范围,并避免在这些点上进行求值,从而避免出现无意义的结果。
其次,我们将研究有理函数的图像和行为。
有理函数的图像通常具有一个或多个垂直渐近线(vertical asymptotes)。
这些渐近线是由分母的根式确定的,因为当分母为零时,有理函数会趋近于正无穷或负无穷。
我们还会研究水平渐近线(horizontal asymptotes),这些线会在无穷远处和有理函数的图像逐渐接近。
另一个重要的概念是有理函数的零点(zeros)和极限(limits)。
有理函数的零点是使得分子为零的输入值,而有理函数的极限是在接近垂直渐近线时的函数值。
我们可以通过求解多项式方程或使用分解法来找到有理函数的零点,并利用极限的概念来研究有理函数在特定点的行为。
在接下来的步骤中,我们将通过实际的案例和问题来应用有理函数的知识。
我们可以使用有理函数来建模各种实际情况,例如经济学中的供需关系、物理学中的运动等。
通过使用有理函数,我们可以对这些实际问题进行定量分析和预测。
最后,我们将总结有理函数的重要性和应用。
有理函数模型的理论及分析

有理函数模型的理论及分析李久飞兰进京【摘要】结合高分辨率遥感影像的处理,探讨了基于有理函数的广义传感器模型RMF,系统地研究了有理函数模型(RFM)的理论、解算过程及其各种特性。
重点阐述了基于正解有理函数模型的地面点定位算法。
通过适当方式构建的有理函数模型其拟合误差可以忽略,可以达到严格成像模型的精度,有能力替代严格成像模型完成摄影测量处理,同时无物理意义的有理函数系数可有效地实现传感器成像参数隐藏。
【期刊名称】《科技创业月刊》【年(卷),期】2010(000)010【总页数】3【关键词】传感器模型;有理函数;定位算法0 引言传统的摄影测量技术利用框幅式相机获取地表影像,依据中心投影性质建立起描述物方空间和像平面之间几何关系的数学模型—共线条件方程。
伴随着遥感技术和航天技术的发展,传感器的构造越来越复杂,探测器件、成像方式发生很大变化,框幅式相机的垄断地位已被打破。
通常的传感器模型都是以共线方程为理论基础,要建立这类严格的传感器模型,必须获取各种成像参数,对航空影像来说包括内方位元素和外方位元素初值,对卫星影像来说包括轨道参数和传感器平台的方位参数以及焦距等。
成像方式各异的传感器带来了新的应用问题,每一种新的传感器面世,都要根据其成像几何专门为其建立数学模型,并在现有的摄影测量软件中加入相应模块进行支持,这就大大增加了实际操作的复杂性和难度。
同时,一些高分辨率商业遥感卫星如IKONOS、QuickBird等的传感器信息暂时并不向用户公开,只向用户提供有理函数模型系数,在不知道其轨道参数和成像有关参数的情况下,使用严格的成像几何模型处理其影像是不可能的。
因此,传感器参数的保密性、成像几何模型的通用性和更高的处理速度要求使用与具体传感器无关的、形式简单的通用成像几何模型取代严格成像几何模型完成遥感影像处理,那么引入一种独立于传感器平台的广义传感器模型是很有意义的。
有理函数模型具有独立于具体传感器、形式简单等特点,能满足传感器参数透明化、成像几何模型通用化和处理高速智能化的要求。
rational functions拟合边缘曲线

我们需要明确什么是有理函数(rational functions)。
有理函数是指两个多项式函数的商,即分子是一个多项式函数,分母是另一个多项式函数。
有理函数的定义域是所有使得分母不等于零的实数。
1. 有理函数的特点有理函数是一类重要的函数,它具有以下几个特点:- 有理函数可以表示一些实际问题中的变化规律,例如经济学中的成本函数、收益函数等都可以用有理函数来表达。
- 有理函数在数学分析中具有重要的地位,它和多项式函数、指数函数、对数函数一样,是一种基本的初等函数。
- 有理函数在工程技术中也有很多应用,比如控制系统中的传递函数、滤波器中的频率特性等都可以用有理函数来描述。
2. 有理函数拟合边缘曲线在实际问题中,很多时候我们需要通过数据来拟合一条曲线,以反映数据的变化规律。
在这个过程中,有理函数常常被用来拟合边缘曲线。
拟合边缘曲线是指通过一组离散的数据点,使用一个数学函数(通常是有理函数)来拟合这些数据点所描述的变化规律。
拟合的目的是找到一个函数,使得该函数与实际数据之间的误差最小化,从而能够较好地预测未知数据点的数值。
3. 有理函数拟合的方法有理函数拟合的方法包括最小二乘法、最大似然估计等。
最小二乘法是一种常用的拟合方法,通过最小化实际数据点与拟合函数之间的误差平方和来确定拟合函数的参数。
最大似然估计则是从统计学角度出发,利用概率分布的最大似然估计方法来确定拟合函数的参数。
4. 实例分析我们以一个实例来说明有理函数拟合边缘曲线的过程。
假设有一组离散的数据点(x1, y1),(x2, y2),……,(xn, yn),我们的目标是找到一个有理函数f(x) = p(x)/q(x)来拟合这些数据点。
我们可以对有理函数f(x)进行参数化的拟合,比如f(x) = (a0 + a1x + a2x^2 + … + amx^m) / (b0 + b1x + b2x^2 + … + bnx^n)。
我们可以利用最小二乘法来确定参数a0, a1, …, am, b0, b1, …, bn的值,从而得到最优的拟合有理函数。
打开matlab拟合工具箱

1.打开CFTOOL工具箱。
在Matlab 6.5以上的环境下,在左下方有一个"Start"按钮,如同Windows的开始菜单,点开它,在目录"Toolboxes"下有一个"Curve Fitting",点开"Curve Fitting Tool",出现数据拟合工具界面,基本上所有的数据拟合和回归分析都可以在这里进行2.2.输入两组向量x,y。
首先在Matlab的命令行输入两个向量,一个向量是你要的x坐标的各个数据,另外一个是你要的y坐标的各个数据。
输入以后假定叫x向量与y向量,可以在workspace里面看见这两个向量,要确保这两个向量的元素数一致,如果不一致的话是不能在工具箱里面进行拟合的。
例如在命令行里输入下列数据:x = [196,186, 137, 136, 122, 122, 71, 71, 70, 33];y = [0.012605; 0.013115; 0.016866; 0.014741; 0.022353; 0.019278; 0.041803; 0.038026;0.038128; 0.088196];3.4. 3.数据的选取。
打开曲线拟合共工具界面,点击最左边的"Data..."按钮,出现一个Data对话框,在Data Sets页面里,在X Data选项中选取x向量,Y Data选项中选取y向量,如果两个向量的元素数相同,那么Create data set按钮就激活了,此时点击它,生成一个数据组,显示在下方Data Sets列表框中。
关闭Data对话框。
此时Curve Fitting Tool 窗口中显示出这一数据组的散点分布图5.6.7.4.曲线拟合(幂函数power)。
点击Fitting...按钮,出现Fitting对话框,Fitting对话框分为两部分,上面为Fit Editor,下面为Table of Fits,有时候窗口界面比较小,Fit Editor部分会被收起来,只要把Table of Fits 上方的横条往下拉就可以看见Fit Editor。
y关于x的函数关系英文

y关于x的函数关系英文1. Function -函数。
2. Relationship -关系。
3. Variable -变量。
4. Dependent variable -因变量。
5. Independent variable -自变量。
6. Equation -方程。
7. Value -值。
8. Domain -域。
9. Range -值域。
10. Slope -斜率。
11. Intercept -截距。
12. Exponential -指数。
13. Logarithmic -对数的。
14. Linear -线性的。
15. Quadratic -二次的。
16. Cubic -三次的。
17. Polynomial -多项式的。
18. Rational -有理的。
19. Radical -根式的。
20. Absolute value -绝对值。
21. Minimum -最小值。
22. Maximum -最大值。
23. Asymptote -渐近线。
24. Intersection -交点。
25. Symmetry -对称性。
26. Conic section -圆锥曲线。
27. Transformation -变换。
例句:1. The relation between x and y can be represented by a function.关于x和y之间的关系可以用一个函数来表示。
2. The value of y is determined by the function relationship with x.y的值由与x的函数关系决定。
3. In this equation, x is the independent variable, while y is the dependent variable.在这个方程中,x是自变量,而y是因变量。
4. The domain of the function is the set of all possible x values.函数的域是所有可能的x值的集合。
高等数学(微积分学)专业术语名词概念定理等英汉对照

高等数学(微积分学)专业术语名词概念定理等英汉对照目录第一部分英汉微积分词汇Part 1 English-Chinese Calculus Vocabulary第一章函数与极限Chapter 1 function and Limi t (1)第二章导数与微分Chapter 2 Derivative and Differential (2)第三章微分中值定理Chapter 3 Mean Value theorem of differentials and the Application of Derivatives (3)第四章不定积分Chapter 4 Indefinite Intergrals (3)第五章定积分Chapter 5 Definite Integral (3)第六章定积分的应用Chapter 6 Application of the Definite Integrals (4)第七章空间解析几何与向量代数Chapter 7 Space Analytic Geomertry and Vector Algebra (4) 第八章多元函数微分法及其应用Chapter 8 Differentiation of functions Several variables and Its Application (5)第九章重积分Multiple Integrals (6)第十章曲线积分与曲面积分Chapter 10 Line(Curve ) Integrals and Surface Integral s (6) 第十一章无穷级数Chapter 11 Infinite Series (6)第十二章微分方程Chapter 12 Differential Equation (7)第二部分定理定义公式的英文表达Part 2 English Expression for Theorem,Definition and Formula第一章函数与极限Chapter 1 Function and Limi t (19)1.1映射与函数(Mapping and Function ) (19)1.2数列的极限(Limit of the Sequence of Number) (20)1.3函数的极限(Limit of Function) (21)1.4无穷小与无穷大(Infinitesimal and Inifinity) (23)1.5极限运算法则(Operation Rule of Limit) (24)1.6极限存在准则两个重要的极限(Rule for theExistence of Limits Two Important Limits) (25)1.7无穷小的比较(The Comparison of infinitesimal) (26)1.8函数的连续性与间断点(Continuity of FunctionAnd Discontinuity Points) (28)1.9连续函数的运酸与初等函数的连续性(OperationOf Continuous Functions and Continuity ofElementary Functions) (28)1.10闭区间上联系汗水的性质(Properties ofContinuous Functions on a Closed Interval) (30)第二章导数与数分Chapter2 Derivative and Differential (31)2.1 导数的概念(The Concept of Derivative) (31)2.2 函数的求导法则(Rules for Finding Derivatives) (33)2.3 高阶导数(Higher-order Derivatives) (34)2.4 隐函数及由参数方程所确定的函数的导数相关变化率(Derivatives ofImplicit Functions and Functions Determined by Parametric Equation andCorrelative Change Rate) (34)2.5 函数的微分(Differential of a Function) (35)第三章微分中值定理与导数的应用Chapter 3 Mean Value Theorem of Differentials and theApplication of Derivatives (36)3.1 微分中值定理(The Mean Value Theorem) (36)3.2 洛必达法则(L’Hopital’s Rule) (38)3.3 泰勒公式(Taylor’s Formula) (41)3.4 函数的单调性和曲线的凹凸性(Monotonicityof Functions and Concavity of Curves) (43)3.5 函数的极值与最大最小值(Extrema, Maximaand Minima of Functions) (46)3.6 函数图形的描绘(Graphing Functions) (49)3.7 曲率(Curvature) (50)3.8 方程的近似解(Solving Equation Numerically) (53)第四章不定积分Chapter 4Indefinite Integrals (54)4.1 不定积分的概念与性质(The Concept andProperties of Indefinite Integrals) (54)4.2 换元积分法(Substitution Rule for Indefinite Integrals) (56)4.3 分部积分法(Integration by Parts) (57)4.4 有理函数的积分(Integration of Rational Functions) (58)第五章定积分Chapter 5 Definite Integrals (61)5.1 定积分的概念和性质(Concept of Definite Integraland its Properties) (61)5.2 微积分基本定理(Fundamental Theorem of Calculus) (67)5.3 定积分的换元法和分部积分法(Integration by Substitution andDefinite Integrals by Parts) (69)5.4 反常积分(Improper Integrals) (70)第六章定积分的应用Chapter 6 Applications of the Definite Integrals (75)6.1 定积分的元素法(The Element Method of Definite Integra (75)6.2 定积分在几何学上的应用(Applications of the DefiniteIntegrals to Geometry) (76)6.3 定积分在物理学上的应用(Applications of the DefiniteIntegrals to Physics) (79)第七章空间解析几何与向量代数Chapter 7 Space Analytic Geometry and Vector Algebar (80)7.1 向量及其线性运算(Vector and Its Linear Operation) (80)7.2 数量积向量积(Dot Product and Cross Product) (86)7.3 曲面及其方程(Surface and Its Equation) (89)7.4 空间曲线及其方程(The Curve in Three-space and Its Equation (91)7.5 平面及其方程(Plane in Space and Its Equation) (93)7.6 空间直线及其方程(Lines in and Their Equations) (95)第八章多元函数微分法及其应用Chapter 8 Differentiation of Functions of SeveralVariables and Its Application (99)8.1 多元函数的基本概念(The Basic Concepts of Functionsof Several Variables) (99)8.2 偏导数(Partial Derivative) (102)8.3 全微分(Total Differential) (103)8.4 链式法则(The Chain Rule) (104)8.5 隐函数的求导公式(Derivative Formula for Implicit Functions). (104)8.6 多元函数微分学的几何应用(Geometric Applications of Differentiationof Ffunctions of Severalvariables) (106)8.7方向导数与梯度(Directional Derivatives and Gradients) (107)8.8多元函数的极值(Extreme Value of Functions of Several Variables) (108)第九章重积分Chapter 9 Multiple Integrals (111)9.1二重积分的概念与性质(The Concept of Double Integralsand Its Properities) (111)9.2二重积分的计算法(Evaluation of double Integrals) (114)9.3三重积分(Triple Integrals) (115)9.4重积分的应用(Applications of Multiple Itegrals) (120)第十章曲线积分与曲面积分Chapte 10 Line Integrals and Surface Integrals (121)10.1 对弧长的曲线积分(line Intergrals with Respect to Arc Length) (121)10.2 对坐标的曲线积分(Line Integrals with respect toCoordinate Variables) (123)10.3 格林公式及其应用(Green's Formula and Its Applications) (124)10.4 对面积的曲面积分(Surface Integrals with Respect to Aarea) (126)10.5 对坐标的曲面积分(Surface Integrals with Respect toCoordinate Variables) (128)10.6 高斯公式通量与散度(Gauss's Formula Flux and Divirgence) (130)10.7 斯托克斯公式环流量与旋度(Stokes's Formula Circulationand Rotation) (131)第十一章无穷级数Chapter 11 Infinite Series (133)11.1 常数项级数的概念与性质(The concept and Properties ofThe Constant series) (133)11.2 常数项级数的审敛法(Test for Convergence of the Constant Series) (137)11.3 幂级数(power Series). (143)11.4 函数展开成幂级数(Represent the Function as Power Series) (148)11.5 函数的幂级数展开式的应用(the Appliacation of the Power Seriesrepresentation of a Function) (148)11.6 函数项级数的一致收敛性及一致收敛级数的基本性质(The UnanimousConvergence of the Series of Functions and Its properties) (149)11.7 傅立叶级数(Fourier Series) (152)11.8 一般周期函数的傅立叶级数(Fourier Series of Periodic Functions) (153)第十二章微分方程Chapter 12 Differential Equation (155)12.1微分方程的基本概念(The Concept of DifferentialEquation) (155)12.2可分离变量的微分方程(Separable Differential Equation) (156)12.3齐次方程(Homogeneous Equation) (156)12.4 一次线性微分方程(Linear Differential Equation of theFirst Order) (157)12.5全微分方程(Total Differential Equation) (158)12.6可降阶的高阶微分方程(Higher-order DifferentialEquation Turned to Lower-order DifferentialEquation) (159)12.7高阶线性微分方程(Linear Differential Equation of HigherOrder) (159)12.8常系数齐次线性微分方程(Homogeneous LinearDifferential Equation with Constant Coefficient) (163)12.9常系数非齐次线性微分方程(Non HomogeneousDifferential Equation with Constant Coefficient) (164)12.10 欧拉方程(Euler Equation) (164)12.11 微分方程的幂级数解法(Power Series Solutionto Differential Equation) (164)第三部分常用数学符号的英文表达Part 3 English Expression of the Mathematical Symbol in Common Use第一部分英汉微积分词汇Part1 English-Chinese Calculus V ocabulary 第一章函数与极限Chapter1 Function and Limit集合set元素element子集subset空集empty set并集union交集intersection差集difference of set基本集basic set补集complement set直积direct product笛卡儿积Cartesian product开区间open interval闭区间closed interval半开区间half open interval有限区间finite interval区间的长度length of an interval无限区间infinite interval领域neighborhood领域的中心centre of a neighborhood领域的半径radius of a neighborhood左领域left neighborhood右领域right neighborhood 映射mappingX到Y的映射mapping of X ontoY 满射surjection单射injection一一映射one-to-one mapping双射bijection算子operator变化transformation函数function逆映射inverse mapping复合映射composite mapping自变量independent variable因变量dependent variable定义域domain函数值value of function函数关系function relation值域range自然定义域natural domain单值函数single valued function多值函数multiple valued function 单值分支one-valued branch函数图形graph of a function绝对值函数absolute value符号函数sigh function整数部分integral part阶梯曲线step curve当且仅当if and only if(iff)分段函数piecewise function上界upper bound下界lower bound有界boundedness无界unbounded函数的单调性monotonicity of a function 单调增加的increasing单调减少的decreasing单调函数monotone function函数的奇偶性parity(odevity) of a function对称symmetry偶函数even function奇函数odd function函数的周期性periodicity of a function周期period反函数inverse function直接函数direct function复合函数composite function中间变量intermediate variable函数的运算operation of function基本初等函数basic elementary function初等函数elementary function幂函数power function指数函数exponential function对数函数logarithmic function三角函数trigonometric function反三角函数inverse trigonometric function 常数函数constant function双曲函数hyperbolic function双曲正弦hyperbolic sine双曲余弦hyperbolic cosine双曲正切hyperbolic tangent反双曲正弦inverse hyperbolic sine反双曲余弦inverse hyperbolic cosine反双曲正切inverse hyperbolic tangent极限limit数列sequence of number收敛convergence收敛于 a converge to a发散divergent极限的唯一性uniqueness of limits收敛数列的有界性boundedness of a convergent sequence子列subsequence函数的极限limits of functions函数()f x当x趋于x0时的极限limit of functions () f x as x approaches x0左极限left limit右极限right limit单侧极限one-sided limits水平渐近线horizontal asymptote无穷小infinitesimal无穷大infinity铅直渐近线vertical asymptote夹逼准则squeeze rule单调数列monotonic sequence高阶无穷小infinitesimal of higher order低阶无穷小infinitesimal of lower order同阶无穷小infinitesimal of the same order 等阶无穷小equivalent infinitesimal函数的连续性continuity of a function增量increment函数()f x在x0连续the function ()f x is continuous at x0左连续left continuous右连续right continuous区间上的连续函数continuous function函数()f x在该区间上连续function ()f x is continuous on an interval不连续点discontinuity point第一类间断点discontinuity point of the first kind第二类间断点discontinuity point of the second kind初等函数的连续性continuity of the elementary functions定义区间defined interval最大值global maximum value (absolute maximum)最小值global minimum value (absolute minimum)零点定理the zero point theorem介值定理intermediate value theorem第二章导数与微分Chapter2 Derivative and Differential速度velocity匀速运动uniform motion平均速度average velocity瞬时速度instantaneous velocity圆的切线tangent line of a circle切线tangent line切线的斜率slope of the tangent line位置函数position function导数derivative可导derivable函数的变化率问题problem of the change rate of a function 导函数derived function左导数left-hand derivative右导数right-hand derivative单侧导数one-sided derivatives()f x在闭区间【a,b】上可导()f x is derivable on the closed interval [a,b]切线方程tangent equation角速度angular velocity成本函数cost function边际成本marginal cost链式法则chain rule隐函数implicit function显函数explicit function二阶函数second derivative三阶导数third derivative高阶导数nth derivative莱布尼茨公式Leibniz formula对数求导法log- derivative参数方程parametric equation相关变化率correlative change rata微分differential可微的differentiable函数的微分differential of function自变量的微分differential of independent variable微商differential quotient间接测量误差indirect measurement error 绝对误差absolute error 相对误差relative error第三章微分中值定理与导数的应用Chapter3 MeanValue Theorem of Differentials and the Application of Derivatives 罗马定理Rolle’s theorem费马引理Fermat’s lemma拉格朗日中值定理Lagrange’s mean value theorem驻点stationary point稳定点stable point临界点critical point辅助函数auxiliary function拉格朗日中值公式Lagrange’s mean value formula柯西中值定理Cauchy’s mean value theorem洛必达法则L’Hospital’s Rule0/0型不定式indeterminate form of type 0/0不定式indeterminate form泰勒中值定理Taylor’s mean value theorem泰勒公式Taylor formula余项remainder term拉格朗日余项Lagrange remainder term 麦克劳林公式Maclaurin’s formula佩亚诺公式Peano remainder term凹凸性concavity凹向上的concave upward, cancave up凹向下的,向上凸的concave downward’concave down拐点inflection point函数的极值extremum of function极大值local(relative) maximum最大值global(absolute) mximum极小值local(relative) minimum最小值global(absolute) minimum目标函数objective function曲率curvature弧微分arc differential平均曲率average curvature曲率园circle of curvature曲率中心center of curvature曲率半径radius of curvature渐屈线evolute渐伸线involute根的隔离isolation of root隔离区间isolation interval切线法tangent line method第四章不定积分Chapter4 Indefinite Integrals原函数primitive function(antiderivative) 积分号sign of integration被积函数integrand积分变量integral variable积分曲线integral curve积分表table of integrals换元积分法integration by substitution分部积分法integration by parts分部积分公式formula of integration by parts有理函数rational function真分式proper fraction假分式improper fraction第五章定积分Chapter5 Definite Integrals曲边梯形trapezoid with曲边curve edge窄矩形narrow rectangle曲边梯形的面积area of trapezoid with curved edge积分下限lower limit of integral积分上限upper limit of integral积分区间integral interval分割partition积分和integral sum可积integrable矩形法rectangle method积分中值定理mean value theorem of integrals函数在区间上的平均值average value of a function on an integvals牛顿-莱布尼茨公式Newton-Leibniz formula微积分基本公式fundamental formula of calculus换元公式formula for integration by substitution 递推公式recurrence formula反常积分improper integral反常积分发散the improper integral is divergent反常积分收敛the improper integral is convergent无穷限的反常积分improper integral on an infinite interval无界函数的反常积分improper integral of unbounded functions绝对收敛absolutely convergent第六章定积分的应用Chapter6 Applications of the Definite Integrals元素法the element method面积元素element of area平面图形的面积area of a luane figure直角坐标又称“笛卡儿坐标(Cartesian coordinates)”极坐标polar coordinates抛物线parabola椭圆ellipse旋转体的面积volume of a solid of rotation旋转椭球体ellipsoid of revolution, ellipsoid of rotation曲线的弧长arc length of acurve可求长的rectifiable光滑smooth功work水压力water pressure引力gravitation变力variable force第七章空间解析几何与向量代数Chapter7 Space Analytic Geometry and Vector Algebra向量vector自由向量free vector单位向量unit vector零向量zero vector相等equal平行parallel向量的线性运算linear poeration of vector 三角法则triangle rule平行四边形法则parallelogram rule交换律commutative law结合律associative law负向量negative vector差difference分配律distributive law空间直角坐标系space rectangular coordinates坐标面coordinate plane卦限octant向量的模modulus of vector向量a与b的夹角angle between vector a and b方向余弦direction cosine方向角direction angle向量在轴上的投影projection of a vector onto an axis数量积,外积,叉积scalar product,dot product,inner product 曲面方程equation for a surface球面sphere旋转曲面surface of revolution母线generating line轴axis圆锥面cone顶点vertex旋转单叶双曲面revolution hyperboloids of one sheet旋转双叶双曲面revolution hyperboloids of two sheets柱面cylindrical surface ,cylinder圆柱面cylindrical surface准线directrix抛物柱面parabolic cylinder二次曲面quadric surface椭圆锥面dlliptic cone椭球面ellipsoid单叶双曲面hyperboloid of one sheet双叶双曲面hyperboloid of two sheets旋转椭球面ellipsoid of revolution椭圆抛物面elliptic paraboloid旋转抛物面paraboloid of revolution双曲抛物面hyperbolic paraboloid马鞍面saddle surface 椭圆柱面elliptic cylinder双曲柱面hyperbolic cylinder抛物柱面parabolic cylinder空间曲线space curve空间曲线的一般方程general form equations of a space curve 空间曲线的参数方程parametric equations of a space curve螺转线spiral螺矩pitch投影柱面projecting cylinder投影projection平面的点法式方程pointnorm form eqyation of a plane法向量normal vector平面的一般方程general form equation of a plane两平面的夹角angle between two planes 点到平面的距离distance from a point to a plane空间直线的一般方程general equation of a line in space方向向量direction vector直线的点向式方程pointdirection form equations of a line方向数direction number直线的参数方程parametric equations of a line两直线的夹角angle between two lines垂直perpendicular直线与平面的夹角angle between a line and a planes平面束pencil of planes平面束的方程equation of a pencil of planes行列式determinant系数行列式coefficient determinant第八章多元函数微分法及其应用Chapter8 Differentiation of Functions of Several Variables and Its Application一元函数function of one variable多元函数function of several variables内点interior point外点exterior point边界点frontier point,boundary point聚点point of accumulation开集openset闭集closed set连通集connected set开区域open region闭区域closed region有界集bounded set无界集unbounded setn维空间n-dimentional space二重极限double limit多元函数的连续性continuity of function of seveal连续函数continuous function不连续点discontinuity point一致连续uniformly continuous偏导数partial derivative对自变量x的偏导数partial derivative with respect to independent variable x高阶偏导数partial derivative of higher order二阶偏导数second order partial derivative 混合偏导数hybrid partial derivative全微分total differential偏增量oartial increment偏微分partial differential全增量total increment可微分differentiable必要条件necessary condition充分条件sufficient condition叠加原理superpostition principle全导数total derivative中间变量intermediate variable隐函数存在定理theorem of the existence of implicit function 曲线的切向量tangent vector of a curve法平面normal plane向量方程vector equation向量值函数vector-valued function切平面tangent plane法线normal line方向导数directional derivative梯度gradient 数量场scalar field梯度场gradient field向量场vector field势场potential field引力场gravitational field引力势gravitational potential曲面在一点的切平面tangent plane to a surface at a point曲线在一点的法线normal line to a surface at a point无条件极值unconditional extreme values 条件极值conditional extreme values拉格朗日乘数法Lagrange multiplier method拉格朗日乘子Lagrange multiplier经验公式empirical formula最小二乘法method of least squares均方误差mean square error第九章重积分Chapter9 Multiple Integrals二重积分double integral可加性additivity累次积分iterated integral体积元素volume element三重积分triple integral直角坐标系中的体积元素volume element in rectangular coordinate system柱面坐标cylindrical coordinates柱面坐标系中的体积元素volume element in cylindrical coordinate system球面坐标spherical coordinates球面坐标系中的体积元素volume element in spherical coordinate system反常二重积分improper double integral曲面的面积area of a surface质心centre of mass静矩static moment密度density形心centroid转动惯量moment of inertia参变量parametric variable第十章曲线积分与曲面积分Chapter10 Line(Curve)Integrals and Surface Integrals对弧长的曲线积分line integrals with respect to arc hength第一类曲线积分line integrals of the first type对坐标的曲线积分line integrals with respect to x,y,and z第二类曲线积分line integrals of the second type有向曲线弧directed arc单连通区域simple connected region复连通区域complex connected region格林公式Green formula第一类曲面积分surface integrals of the first type对面的曲面积分surface integrals with respect to area有向曲面directed surface对坐标的曲面积分surface integrals with respect to coordinate elements第二类曲面积分surface integrals of the second type有向曲面元element of directed surface高斯公式gauss formula拉普拉斯算子Laplace operator格林第一公式Green’s first formula通量flux散度divergence斯托克斯公式Stokes formula环流量circulation旋度rotation,curl第十一章无穷级数Chapter11 Infinite Series一般项general term部分和partial sum余项remainder term等比级数geometric series几何级数geometric series公比common ratio调和级数harmonic series柯西收敛准则Cauchy convergence criteria, Cauchy criteria for convergence正项级数series of positive terms达朗贝尔判别法D’Alembert test柯西判别法Cauchy test 交错级数alternating series绝对收敛absolutely convergent条件收敛conditionally convergent柯西乘积Cauchy product函数项级数series of functions发散点point of divergence收敛点point of convergence收敛域convergence domain和函数sum function幂级数power series幂级数的系数coeffcients of power series 阿贝尔定理Abel Theorem收敛半径radius of convergence收敛区间interval of convergence泰勒级数Taylor series麦克劳林级数Maclaurin series二项展开式binomial expansion近似计算approximate calculation舍入误差round-off error,rounding error欧拉公式Euler’s formula魏尔斯特拉丝判别法Weierstrass test三角级数trigonometric series振幅amplitude角频率angular frequency初相initial phase矩形波square wave谐波分析harmonic analysis直流分量direct component基波fundamental wave二次谐波second harmonic三角函数系trigonometric function system 傅立叶系数Fourier coefficient傅立叶级数Forrier series周期延拓periodic prolongation正弦级数sine series余弦级数cosine series奇延拓odd prolongation偶延拓even prolongation傅立叶级数的复数形式complex form of Fourier series第十二章微分方程Chapter12 Differential Equation解微分方程solve a dirrerential equation 常微分方程ordinary differential equation偏微分方程partial differential equation,PDE微分方程的阶order of a differential equation微分方程的解solution of a differential equation微分方程的通解general solution of a differential equation初始条件initial condition微分方程的特解particular solution of a differential equation 初值问题initial value problem微分方程的积分曲线integral curve of a differential equation 可分离变量的微分方程variable separable differential equation 隐式解implicit solution隐式通解inplicit general solution衰变系数decay coefficient衰变decay齐次方程homogeneous equation一阶线性方程linear differential equation of first order非齐次non-homogeneous齐次线性方程homogeneous linear equation非齐次线性方程non-homogeneous linear equation常数变易法method of variation of constant暂态电流transient stata current稳态电流steady state current伯努利方程Bernoulli equation全微分方程total differential equation积分因子integrating factor高阶微分方程differential equation of higher order悬链线catenary高阶线性微分方程linera differential equation of higher order 自由振动的微分方程differential equation of free vibration强迫振动的微分方程differential equation of forced oscillation 串联电路的振荡方程oscillation equation of series circuit二阶线性微分方程second order linera differential equation线性相关linearly dependence线性无关linearly independce二阶常系数齐次线性微分方程second order homogeneour linear differential equation with constant coefficient二阶变系数齐次线性微分方程second order homogeneous linear differential equation with variable coefficient特征方程characteristic equation无阻尼自由振动的微分方程differential equation of free vibration with zero damping 固有频率natural frequency 简谐振动simple harmonic oscillation,simple harmonic vibration微分算子differential operator待定系数法method of undetermined coefficient共振现象resonance phenomenon欧拉方程Euler equation幂级数解法power series solution数值解法numerial solution勒让德方程Legendre equation微分方程组system of differential equations常系数线性微分方程组system of linera differential equations with constant coefficient第二部分定理定义公式的英文表达Part2 English Expression for Theorem, Definition and Formula第一章函数与极限Chapter 1 Function and Limit1.1 映射与函数 (Mapping and Function)一、集合 (Set)二、映射 (Mapping)映射概念 (The Concept of Mapping) 设X , Y 是两个非空集合 , 如果存在一个法则f ,使得对X 中每个元素x ,按法则f ,在Y 中有唯一确定的元素y 与之对应 , 则称f 为从X 到 Y 的映射 , 记作:f X Y →。
maple函数大全
rtable data structures
序包
Matlab
Matlab Link
与 Matlab 的接口函数
Ore_algebra
Bacic calculations in algebras of 线性算的基本代数运算
linear onerators
PDEtools
tools for solveing partial differential 偏微分方程相关函数
雅可比椭圆函数
雅可比椭圆函数雅
雅可比θ函数
JacobiZeta KelvinBei KelvinBer KelvinHei KelvinHer
雅可比ζ函数 开尔文 Bei 函数 开尔文 Her 函数 开尔文 Hei 函数 开尔文 Her 函数
KelvinKei KelvinHer KummerM KummerU LanbertW Lcm LegendreP LegendreQ Lerchphi Li Linsolve Lommels1 Lommels2 MOLS Maple_floats MatlabMatrix MeijerG 函数名称 Normal Nullspace Power Powmod Prem Primitive Primpart 函数名称
多项式可分解为同阶因式的可能性 Product(乘)函数的简化形式 双γ函数与多γ函数 Quo(求多项式的商)函数的简化形式 一种递归方程解的数据结构 有限域的随机多项式 有限域的随机 monic prime 多项式 Ratrecon(重组有理函数)的简化形式 取复数的实部 rem(求多项式相除余项)函数的简化形式 Resultant(合成多项式)函数简化形式 求方程的根 多项式求模后的根 Sperm(异伪余式)函数的简化形式 查找文本 双曲正弦函数 正弦积分 矩阵的 Smith 正交化 非平方因式分解 转移正弦积分 StruveH 函数 StruveL 函数 sum(求和)和函数的简化形式 基本功能 计算矩阵的奇异数/向量 显示文本
maple函数大全
复合函数
combinatorial structures
复合结构
context sensitive menus
上下文敏感菜单
differential algebra
偏微分代数
differential forms
微分形式
financial mathematics
金融数学
rational generating functions 有理数产生函数
assign assigned assume asympt attribute bernstein branches bspline cat ceil charfcn chrem close coeff compiletable compoly conjugate content convergs clnvert copy cos cosh cot coth csc csch cdgn currentdir dawson 函数名称 define degree denom depends diff diffop dilog dinterp disassemble discont discrim dismantle
查找文本 双曲正弦函数 正弦积分 矩阵的 Smith 正交化 非平方因式分解 转移正弦积分 StruveH 函数 StruveL 函数 sum(求和)和函数的简化形式 基本功能 计算矩阵的奇异数/向量 显示文本 韦伯函数 Weierstrass P 函数 Weierstrass P 函数的导数 WeierstrassΣ函数 Weierstrassζ函数 Whittaker M 函数 Whittaker W 函数 黎曼ζ函数 绝对值 加法 添加坐标系 得到所指表达式的内存地址 对多项式子的替换 定义缩写 求出等式的全部可能解 顺序显示已定义的变量名 反对称参数 应用运算规则 反余弦函数 反双曲余弦函数 反余切函数 反双曲余切函数 反正割函数 反双曲正割函数 反正弦函数 反双曲正弦函数 反正切函数 反双曲正切函数 求复数坐标下的向量角度 基本功能 定义数组
高等数学方明亮44几种特殊类型函数的积分.ppt

,
1 A(1 x2 ) (Bx C)(1 2x),
整理得 1 ( A 2B)x2 (B 2C)x C A,
A 2B 0,
B A
(1
2C 0, C 1,
1 2x)(1
x2)
A 4, 5 4
5 1 2
B 2,C 52xx来自5 1 x21 5
1 5.
,
2024年9月27日星期五
一、 有理函数的积分
(Integration of Rational Function)
有理函数的定义:两个多项式的商表示的函数.
P(x) Q( x)
a0 xn b0 x m
a1 x n1 b1 x m1
an1 x an bm1 x bm
其中m、n都是非负整数;a0 ,a1 ,,an及b0 ,b1,,bm 都是实数,并且a0 0,b0 0.
1 6a3
ln
x3 a3 x3 a3
C
(2) 原式
sin2 x sin3
x
cos2 cos x
x
dx
dx sin x cos x
cos sin 3
x x
dx
d tan x tan x
d sin sin 3
x x
2024年9月27日星期五
29
目录
上页
下页
返回
2. 求
(a
sin
x
1 b
解法 2 令
a sin ,
a2 b2
b cos
a2 b2
原式
a2
1
b2
dx
cos2 (x )
a
2
1
b2
tan(x
)
数学专业英语词汇(F)

数学专业英语词汇(F)f distribution f分布f ratio 方差比f space f空间f test f检定face 面face centered 面心的face centered cubic 面心立方体的face of a polyhedron 多面体面face operator 面算子factor 因子factor analysis 因子分析factor combination 因子组合factor group 商群factor groupoid 商广群factor loading 因子载荷factor matrix 因子矩阵factor model 因子模型factor module 商模factor of a polynomial 多项式的因子factor of proportionality 比例系数factor out 提出撰因子factor ring 商环factor set 商集factor space 商空间factor system 因子组factor theorem 因子定理factorability 因子可分解性factorable 可因子分解的factorial 阶乘factorial cumulant 阶乘累积量factorial design 因子设计factorial experiment 析因实验factorial notation 阶乘记号factorial of an integer 整数的阶乘factorial polynomial 阶乘多项式factorial series 阶乘级数factoring 因式分解factorizable 可因子分解的factorization 因式分解factorization method 因子分解方法factorization theorem 因子分解定理factorize 因子分解fair game 适当对策faithful anti representation 一一反表示faithful functor 一一的函子faithful module 一一的模faithful representation 一一表示faithfully flat ring 一一平坦环false 假的false conclusion 假结论family 族family of curves 曲线族family of elements 元素族family of functions 函数族family of orthogonal curves 正交曲线族family of planes 平面族family of sets 集族family of solutions 解族family of surfaces 曲面族fan 扇形farey series 法雷级数fast fourier transformation 快速傅里叶变换fatou lemma 法都引理favorable case 有利情形favorable event 有利事件feasible base 可行基feasible constraint 可行约束feasible control 可行控制feasible direction 可行方向feasible point 可行点feasible region 可行区域feasible restriction 可行约束feasible solution 可行解feeble solution 弱解fermat last theorem 费马最后定理fermat number 费马数fermat spiral 费马螺线fermat theorem 费马定理feynman integral 费曼积分fiber 纤维fiber bundle 纤维丛fiber map 纤维映射fiber preserving mapping 保纤映射fiber space 纤维空间fibonacci number 斐波那契数fibring 纤维空间fiducial distribution 置信分布fiducial estimation 置信估计fiducial inference 置信推理fiducial limit 置信限fiducial probability 置信概率fiducial region 置信区域field 域;场field of algebraic functions 代数函数域field of constants 常数域field of definition 定义域field of events 事件场field of extremals 极值曲线场field of forces 力场field of numbers 数域field of rational functions 有理函数域field of rationals 有理数域field of real numbers 实数域field of scalars 系数域field of sets 集域field theory 域论;场论figure 图形filter 滤子filter base 滤子基filtered category 滤子化范畴filtered degree 滤子化次数filtered group 过滤群filtering 过滤filtration 过滤final decision 最后判决final functor 尾函子final state 终态final topology 终拓扑fine sheaf 强层fine topology 细拓扑fineness 细度finer topology 较细拓扑finish time 终止时间finite automaton 有限自动机finite base 有限基finite cardinal 有限基数finite constructibility 有限可构成性finite continued fraction 有限连分数finite cyclic group 有限循环群finite definability 有限可定义性finite difference approximation 有限差分逼近finite difference equation 有限差分方程finite dimensional 有限维的finite dimensional extension field 有限维扩张域finite dimensional operator 有限维算子finite discontinuity 有限不连续性finite element method 有限元法finite equation 有限方程finite extension 有限维扩张域finite field 有限域finite function 有限函数finite game 有限对策finite group 有限群finite induction 有限归纳法finite mathematics 有限数学finite measure 有限测度finite model 有限模型finite multiplier 有限乘数finite nilpotent group 有限幂零群finite number plane 有限数平面finite ordinal number 有限序数finite part 有限部分finite partition 有限划分finite point 有限点finite presentation 有限表示finite progression 有限级数finite quantity 有限量finite sequence 有限序列finite series 有限级数finite set 有限集finite sum 有限和finite valued function 有限值函数finitely additive 有限加性的finitely additive measure 有限加性测度finitely cocomplete category 有限共完全范畴finitely complete category 有限完全范畴finitely generatable vector space 有限可生成向量空间finitely generated abelian group 有限生成阿贝耳群finitely generated algebra 有限生成代数finitely generated extension field 有限生成扩张域finitely generated group 有限生成群finitely generated ideal 有限生成理想finitely generated module 有限生成模finitely presented 有限出现的finitely presented group 有限出现群finitely representable 有限可表示的finitely valued function 有限值函数finiteness 有限性finiteness principle 有限性原理finiteness theorem 有限性原理finsler manifold 芬斯莱廖first approximation 首次近似first axiom of countability 第一可数性公理first boundary condition 狄利克雷边界条件first boundary value problem 狄利克雷问题first class 第一类first comparison test 第一比较检验first derivative 一阶导数first fundamental form 第一基本形式first fundamental form of surface 曲面的第一基本形式first integral 初积分first isomorphism theorem 同态定理first mean value theorem 平均值定理first obstruction 第一障碍类first order theory 一阶理论first quadrant 第一象限first quartile 第一四分位数first term 首项first theorem of the mean 第一平均值定理fisher z distribution 费歇耳z分布fitted curve 拟合曲线fitting 拟合five color theorem 五色定理five figure 五位数的five point finite difference scheme 五点有限差分格式five sided 五面的fix 固定fixed element 不变元fixed error 偏倚fixed point 定点fixed point calculation 定点计算fixed point method 不动点法fixed point of mapping 映射的不动点fixed point representation 定点表示法fixed point theorem 不动点定理fixed sample 固定样本fixed vector 固定向量flabby resolution 松弛分解flabby sheaf 散射层flag 旗flag manifold 旗廖flat morphism 平坦射flat point 平坦点flat space 平坦空间flat surface 平面flatness 平坦性flecnode 拐结点flex 拐点floating point 浮点floating point calculation 浮点记数法floating point notation 浮点记数法floating point representation 浮点记数法flow 流flow line 吝flow of scalar field 纯量场的流flow problem 潦题fluctuation 起状fluent 变数fluid 铃fluid dynamics 铃动力学fluid mechanics 铃力学flux 量flux of the vector 量fluxion 导数focal axis 焦轴focal chord 焦弦focal circle 焦圆focal conic 焦点圆锥曲线focal distance 焦距focal length 焦距focal line 焦线focal plane 焦平面focal point 焦点focal radius 焦半径focal strip 焦带focal surface 焦曲面focus 焦点focus of a parabola 抛物线的焦点fold singularity 折奇性folding 卷积foliated manifold 叶状廖foliation 叶状结构folium 叶形线folium of descartes 笛卡儿叶形线follow 跟foot 垂足foot of a perpendicular 垂足force 力force function 力函数force of gravity 重力force of inertia 惯性力force polygon 力的多角形force triangle 力三角形force vector 力向量forced oscillation 受迫振动forced vibration 受迫振动forcing method 力迫法forecasting 预报form 形式formal 形式的formal derivative 形式导数formal language 形式语言formal logic 形式逻辑formal model 形式模型formal power series 形式幂级数formal system 形式系统formally real field 形式实域formation 形成formation rule 形成规则formless 无形状的formula 公式formula language 公式语言formula of computation 计算公式formula of propositional logic 命题逻辑公式formulate 公式化formulation 用公式表示forward difference 前向差分forward difference operator 前向差分算子forward difference quotient 前向差商forward solution 前向解法foundation 基础foundation of geometry 几何基础foundations of geometry 几何基础foundations of mathematics 数学基础four address 四地址的four address instruction 四地址指令four color conjecture 四色猜想four color problem 四色问题four color theorem 四色定理four digit 四位的four dimensional 四维的four dimensionality 四维性four fold table 四重表four vector 四元向量four vertex theorem 四顶点定理fourier analysis 傅里叶分析fourier analyzer 傅里叶分析仪fourier bessel series 傅里叶贝塞耳级数fourier bessel transformation 汉克尔变换fourier coefficient 傅里叶系数fourier cosine transform 傅里叶余弦变换fourier expansion 傅里叶展开fourier integral 傅里叶积分fourier integral equation 傅里叶积分方程fourier integral theorem 傅里叶积分定理fourier kernel 傅里叶核fourier series 傅里叶级数fourier sine transform 傅里叶正弦变换fourier synthesis 傅里叶综合法fourier transform 傅里叶变换fourierstieltjes transform 傅里叶斯蒂尔吉斯变换fractal 分形fractal dimension 分形维数fraction 分数fraction in lowest terms 最简分数fractional derivative 分数导数fractional equation 分数方程fractional exponent 分式指数fractional function 分数函数fractional ideal 分式理想fractional integral 分数次积分fractional number 分数fractional part 分数部分fractional rational function 分数有理函数fractional replication 分数配置fractionary ideal 分式理想frame 架frechet derivative 弗雷谢导数frechet differentiable function 弗莱谢可微函数frechet differential 弗雷谢微分frechet space f空间fredholm alternative 弗雷德霍姆择一fredholm alternative theorem 弗雷德霍姆择一定理fredholm determinant 弗雷德霍姆行列式fredholm integral equation 弗雷德霍姆积分方程fredholm operator 弗雷德霍姆算子fredholm point 弗雷德霍姆点fredholm radius 弗雷德霍姆半径fredholm type integral equation 弗雷德霍姆型积分方程free decision variable 自由决策变量free end 自由端free group 自由群free groupoid 自由广群free index 自由指标free liberty 自由可动性free mobility 自由可动性free monoid 自由独异点free optimization problem 自由最优化问题free product 自由积free semigroup 自由半群free subscript 自由添标free ultrafilter 自由超滤子free variable 自由变量free vector 自由向量frequency 频率frequency curve 频率曲线frequency diagram 频率图frequency distribution 频率分布frequency function 频率函数frequency polygon 频数多边形frequency theory of probability 概率的频率论fresnel integral 菲涅耳积分friction 摩擦frobenius algebra 弗罗宾尼斯代数frobenius automorphism 弗罗宾尼斯自同构frobenius group 弗罗宾尼斯群front 前面frontier 边界frontier point 边界点frustum of a paraboloid 平截头抛物面体frustum of cone 平截头圆锥体frustum of pyramid 截棱锥fubini theorem 富比尼定理fuchsian function 富克斯函数fuchsian group 富克斯群fulcrum 支点full inhomogeneous lorentz group 完全非齐次洛伦茨群full line 实线full linear group 全线性群full solid angle 完全立体角full subcategory 完全子范畴full unimodular group 完全幺模群fully faithful 完全一一的fully faithful functor 完全一一函子fully normal space 仿紧空间fully reducible star body 完全可约星形体fully transitive group 全可迁群function 函数function algebra 函数代数function chart 函数尺function code 操纂function constant 函数常数function continuous on the right 右连续函数function field 函数域function of bounded variation 有界变差函数function of class 类函数function of complex variable 复变函数function of confluent type 合镣函数function of function 合成函数function of n variables n元函数function of one variable 一元函数function of several real variables 多实元函数function of several variables 多元函数function of third order 三阶函数function of unbounded variation 无界变差函数function representation 函数表示function sequence 函数序列function series 函数级数function space 函数空间function symbol of n arguments n变数函数符号function theoretic 函数论的function theoretic null set 函数论的零集function theory 函数论function value 函数值function variable 函数变数functional 泛函functional analysis 泛函分析functional calculus 函项演算functional constant 函数常数functional dependence 函数相关functional determinant 函数行列式functional differential equation 泛函微分方程functional equation 函数方程functional expression 函数式functional matrix 函数矩阵functional relation 函数关系functional scale 函数尺functional space 函数空间functional symbol 函数符号functional transformation 泛函变换functionelement 函数元素functor 函子fundamental 基本的fundamental class 基本类fundamental cocycle 基本上循环fundamental conjunction 基本合取fundamental constants 基本常数fundamental curve 基本曲线fundamental cycle 基本闭链fundamental determinant 基本行列式fundamental discriminant 基本判别式fundamental domain 基本域fundamental element 基本元素fundamental equations 基本方程fundamental form 基本形式fundamental formulae 基本公式fundamental frequency 基频fundamental function 特寨数fundamental group 基本群fundamental groupoid 基本广群fundamental homology class 基本同掂fundamental identity 基本恒等式fundamental invariant 基本不变量fundamental law 基本律fundamental lemma 基本引理fundamental lemma of calculus of variation 变分法的基本引理fundamental matrix 基本矩阵fundamental net 基本有向点族fundamental parallelepipedon 基本平行六面体fundamental period 基本周期fundamental period parallellogram 基本周期平行四边形fundamental period parallelogram 原始周期平行四边形fundamental point 基本点fundamental polygon 基本多角形fundamental sequence 柯悟列fundamental set 基本集fundamental solution 基本解fundamental surface 基本曲面fundamental system of solutions 基本解组fundamental tensor 基本张量fundamental tetrahedron 基本四面体fundamental theorem 基本定理fundamental theorem of algebra 代数基本定理fundamental theorem of calculus 微积分基本定理fundamental transformation 基本变换fundamental unit 基本单位fundamentals 原理fuzzy set 模糊集。
Matlab主要函数使用方法

Matlab主要函数使用方法ndims(A)用ndims命令常看矩阵的维数1. matlab中mod的用法简单的说mod(a,b)就是求的是a除以b的余数。
比方说mod(100,3)=1,mod(17,6)=5Examplesmod(13,5)ans =3mod([1:5],3)ans =1 2 0 1 2mod(magic(3),3) magic(n):其元素由1到n的自然数组成,每行、每列及两对角线的元素和均为(n的三次方+n)/2ans =2 1 00 2 11 0 22. 关于Matlab中size()的问题size按照下面的形式使用:[m,n]=size(a,x)。
一般的,函数的输入参量x不是用,这是当只有一个输出变量时,size返回一个行向量,第一个数为行数,第二个数为列数;如果有两个输出变量,第一个返回量为行数,第二个返回数为列数。
当使用x时,x=1返回行数,x=2返回列数,这时只有一个返回值。
我要问的是:I=im2double(imread(file)) ;//把图像数组转换成double精度类型;if(size(I,3) > 1)I = rgb2gray( I ) ;%把图片转为灰度图片end中size(I,3) 代表什么意思?按照上面的说法X=1,2都可以理解。
I 代表的是图像数组阿,不是二维的么?那么size(I,3) 中的3 代表什么?一个函数可以是多维的..比如说是一个10*10*10*10的距阵那就是说这个距阵是4维的..那个'()'里的3就是指的第三维3.r = corr2(A,B)计算两个矩阵的二维相关系数.4.matlab中imshow显示问题各位好,将一幅灰度图像经过小波变换后,数据变成double型,对变换后的图像进行显示时,设变换后的图像为y,为何用imshow(uint8(y))和imshow(y,[])时的图像显示结果不同呢?请问该如何正确显示变换后的图像呢?着急解决,请各位xdjm帮帮忙吧!回答:imshow(uint8(y))是按照256级灰度显示y的绝对数据。
